1
Nonlinear 1 ono one pera ors
.

\ ()}
,
Springer- erlag

Nonlinear Functional Analysis
and its Applications
I 1/ B: Nonlinear Monotone Operators

David Hilbert (1862-1943)

Eberhard Zeidler
Nonlinear
Functional Analysis
and its Applications
II/B: Nonlinear Monotone Operators
Translated by the Author and by Leo F. Boront
With 74 Illustrations
Springer- Verlag
New York Berlin Heidelberg
London Paris Tokyo

Eberhard Zeidler
Scktion Mathematik
Karl-Marx-Platz
70 10 lei pzig
German Democratic Republic
Leo .. 8oront
Department of Mathematics
University of Idaho
Moscow, ID 83843
U.S.A.
Mathematics Subject Classification (1980): 46xx
Library of Congress Cataloging in Publication Dara
(Revised for vol. 2 pts. A-B)
Zeidler, Eberhard.
Vol. 2. pts. A- B: Translated by the author and
Leo L. Boron.
Vol. 3: Translated by Leo L. Boron.
Vol. 4: Translated by Juergen Quandt.
Includes bibliographies and indexes.
Contents: I. Fixed point theorems - 2. pt A. Linear
monotone operators. Pt. B Nonlinear operators - [etc.)
- 4. Applications to mathematical physics.
1. Nonlinear functional analysis. I. Title.
QA321.5.1A513 1985 515.7 83-20455
Printed on acid-free paper
Previous edition. Vorlesungen uher nichtlineare FUllkt;onala,uJ/ys;s. V ols. I III, published by
B. G. Teubner Verlagsgesellschaft. 7010 Leipzig. Sternwartenstrasse 8. Deutsche Demokrati
Republik.
@ 1990 by Springer-Verlag New York Inc.
All nghts reserved. This work may not be translated or copied In whole or in part without the
wrillen permission of the publisher (Springer- Verlag, 175 Fifth A venue. New York. New York
10010, U.S.A.), excepl for bncf cx<.-erpts in conneclion with reviews or scholarly analysis. Use in
connection with any form of information slorag and relrieval. electronic adaptation. computer
software, or by similar or dissimilar methodology now known or hereafter developed is forbidden.
Typeset by Asco Trade Typesetting LId.. JIong Kong.
Printed and bound by Edwards Brothers, Inc., AM Arbor, Michigan
Printed in Ihe United Slaies of America.
9 8 7 6 5 4 3 2 I
ISBN o-381-91167-X Springer-rlag New York Berlin Heidelberg
ISBN 3-540-91167-X Springer-rlag New York Berlin Heidelberg

To the mentor}t of mJ' parents


Preface to Part II/B
The present book is part of a comprehensive exposition of the main principles
of nonlinear functional analysis and its numerous applications to the natural
sciences and mathematical economics. The presentation is self-contained and
accessible to a broader audience of mathematicians, natural scientists. and
engineers. The material is organized as follows:
Part I: Fixed-point theorems.
Part II: Monotone operators.
Part III: Variational methods and optimization.
Parts IV IV: Applications to mathematical physics.
Here, Part II is divided into two subvolumes:
Part II/A: Linear monotone operators.
Part IliB: Nonlinear monotone operators.
These two subvolumes form a unit equipped with a uniform pagination. The
contents of Parts 11/ A and II/B and the basic strategies of our presentation
have been discussed in detail in the Preface to Part II/A. The present volume
contains the complete index material for Parts IliA and II/B.
F'or valuable hints I would like to thank Ina Letzel, Frank Benkert, Werner
Berndt, Gunther Berger, Hans-Peter Gittel, Matthias Gunther, Jurgen Herrler,
and Rainer Schumann. I would also like to thank Professor Stefan Hildebrandt
for his generous hospitality at the SFB in Bonn during several visits .in the
last few years. In conclusion, I would like to thank Springer-Verlag for a
harmonious collaboration.
Leipzig
Summer 1989
Eberhard Zeidler
. .
VII


Contents (Part II/B)
Preface to Part II/B
GENERALIZATION TO NONLINEAR
STATIONARY PROBLEMS
Basic Ideas of the Theory of Monotone Operators
("'HAPTER 25
Lipschitz Continuous, Strongly Monotone Operators, the
Projection -Iteration Method, and Monotone Potential Operators
25.1. Sequences of k-Contractive Operators
25.2. The Projection Iteration Method for k-Contractivc Operators
25.3. Monotone Operators
25.4. The Main Theorem on Strongly Monotone Operators, and
the Projection Iteration Method
25.5. Monotone and Pseudomonotone Operators, and
the Calculus of Variations
9:!5.6. The Main Theorem on Monotone Potential Operators
25.7. The Main Theorem on Pseudomonotone Potential Operators
25.8. Application to the Main Theorem on Quadratic Variational
Inequalities
25.9. Application to Nonlinear Stationary Conservation Laws
25.IO. Projection Iteration Method for Conservation Laws
25.11. The Main Theorem on Nonlinear Stationary Conservation Laws
25.12. Duality Theory for Conservation Laws and Two-sided
a posteriori Error Estimates for the Ritz Method
25.13. The Kacanov Method for Stationary Conservation Laws
25.14. The Abstract Kacanov Method for Variational Inequalities
. .
VII
469
471
495
497
499
500
503
506
516
518
519
521
527
535
537
542
545
IV

x
Contents (Part II/B)
CHAPTER 26
Monotone Operators and Quasi-Linear Elliptic
Differential Equations 553
26.1. Hemlcontinuity and Demicontinuity 554
26.2. · The Main Theorem on Monotone Operators 556
26.3. The Nemyckii Operator 561
26.4. Generalized Gradient Method for the Solution of
the Galerkin Equations 564
26.5. Application to Quasi-Linear Elliptic Differential Equations
of Order 2m 567
26.6. Proper Monotone Operators and Proper Quasi-linear Elliptic
Differential Operators 576
CHAPTER 27
Pseudomonotone Operators and Quasi-linear Elliptic
Differential Equations 580
27.1. The Conditions (M) and (S), and the Convergence of
the Galerkin Method 583
27.2. Pseudomonotone Operators 585
27.3. The Main Theorem on Pseudomonotone Operators 589
27.4. Application to Quasi-linear Elliptic Differential Equations 590
27.5. Relations Between Important Properties of Nonlinear Operators 595
27.6. Dual Pairs of B-Spaces 598
27.7. The Main Theorem on Locally Coercive Operators 598
27.8. Application to Strongly Nonlinear Differential Equations 604
CHAPTER 28
Monotone Operators and Hammerstein Integral Equations 615
28.1. A Factorization Theorem for Angle-Bounded Operators 619
28.2. Abstract Hammerstein Equations with Angle-Bounded
Kernel Operators 620
28.3. Abstract Hammerstein Equations with Compact Kernel Operators 625
28.4. Application to Hammerstein Integral Equations 627
28.5. Application to Semilinear Elliptic Differential Equations 632
CHAPTER 29
Noncoercive Equations, Nonlinear Fredholm Alternatives,
Locally Monotone Operators, Stability, and Bifurcation 639
29.1. Pseudoresolvent, Equivalent Coincidence Problems, and the
Coincidence Degree 643
29.2. "redholm Alternatives for Asymptotically Linear, Compact
Perturbations of the Identity 650
29.3. Application to Nonlinear Systems of Real Equations 652
29.4. Application to Integral Equations 653
29.5. Application to Differential Equations 653
929.6. The Generalized Antipodal Theorem 654
29. 7. Fredholm Alternatives for Asymptotically linear (S)-Operators 657
29.8. Weak Asymptotes and Fredholm Alternatives 657

Contents (Part II/B)
.
XI
29.9. Application to Semilinear Elliptic Differential Equations of
the Landesman - Lazer Type 661
29.IO. The Main Theorem on Nonlinear Proper Fredholm Operators 665
29.11. Locally Strictly Monotone Operators 677
29.12. Locally Regularly Monotone Operators. Minima, and Stability 679
29.13. Application to the Buckling of Beams 697
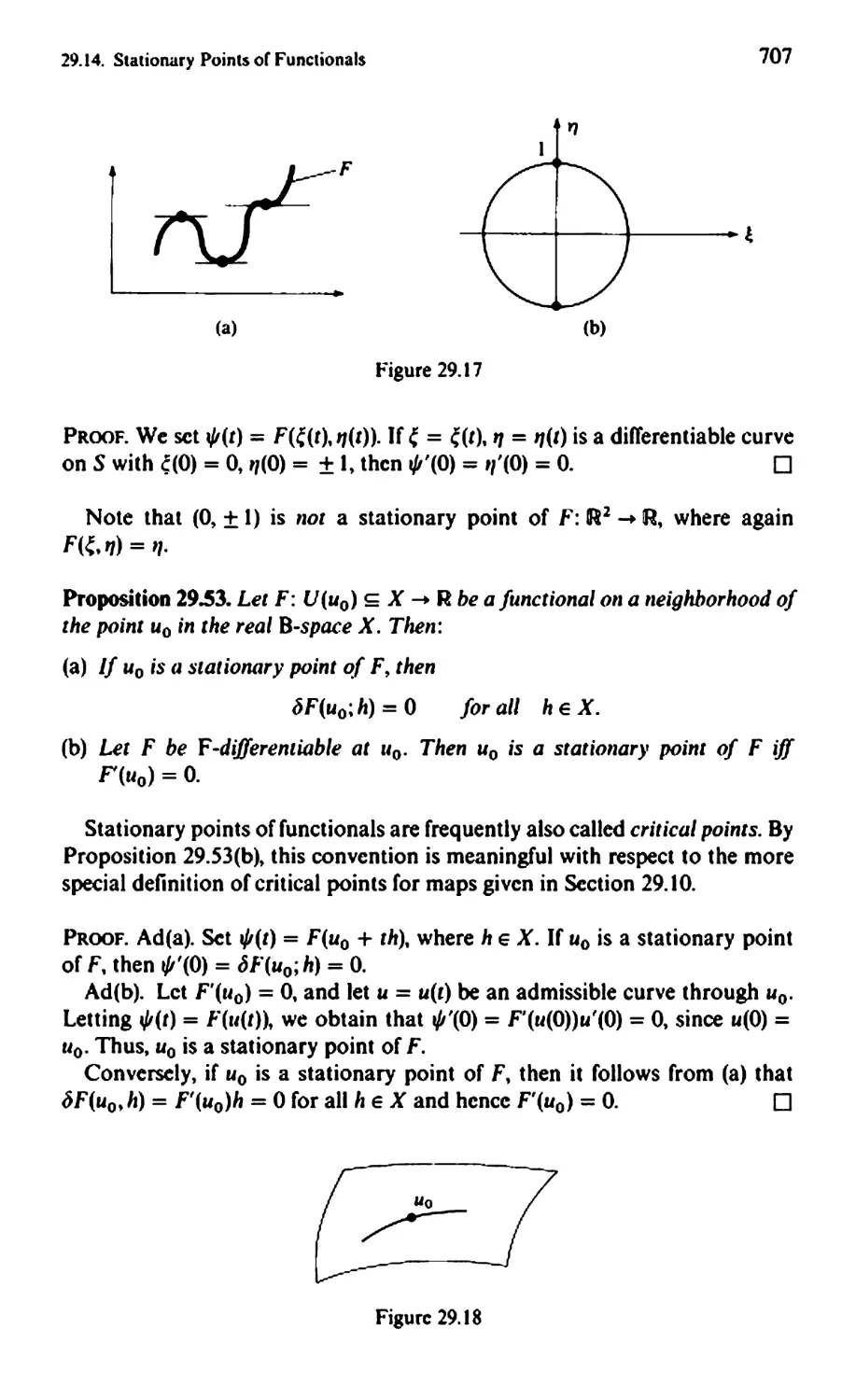
29.14. Stationary Points of Functionals 706
29.15. Application to the Principle of Stationary Action 708
*29.16. Abstract Statical Stability Theory 709
29.17. The Continuation Method 712
29.18. The Main Theorem of Bifurcation Theory for Fredholm
Operators of Variational Type 712
29.19. Application to the Calculus of Variations 722
29.20. A General Bifurcation Theorem for the Euler Equations
and Stability 730
29.21. A Local Multiplicity Theorem 733
29.22. A Global Multiplicity Theorem 735
GENERALIZATION TO NONLINEAR
NONSTATIONARY PROBLEMS 765
CHAPTER 30
First-Order Evolution Equations and the Galerkin Method 767
30.I, Equivalent J."ormulations of First-Order Evolution Equations 767
*30,2. The Main Theorem on Monotone F'irst-Order Evolution Equations 770
30.3. Proof of the Main Theorem 771
30.4, Application to Quasi-Linear Parabolic Differential Equations
of Order 2m 779
30.5, The Main Theorem on Semibounded Nonlinear
Evolution Equations 783
30,6. Application to the Generalized Korteweg de Vries Equation 790
CHAPTER 31
Maximal Accretive Operators, Nonlinear Nonexpansive Semigroups,
and "irst-Order Evolution Equations 817
31.1. The Main Theorem 819
31.2. Maximal Accretive Operator 820
31,3. Proof of the Main Theorem 822
31.4. Application to Monotone Coercive Operators on B-Spaces 827
31.5. Application to Quasi-Linear Parabolic Differential Equations 829
31.6. A Look at Quasi-Linear Evolution Equations 830
31. 7. A Look at Quasi-Linear Parabolic Systems Regarded as
Dynamical Systems 832
CHAPTER 32
Maximal Monotone Mappings 840
]2,I, Basic Ideas 843
J2.2. Definition of Maximal Monotone Mappings 850

. .
XII
Contents (Part II/B)
932.3. Typical Examples for Maximal Monotone Mappings 854
32.4. The Main Theorem on Pseudomonotone Perturbations of
Maximal Monotone Mappings 866
32.5. Application to Abstract Hammerstein Equations 873
932.6. Application to Hammerstein Integral Equations 874
32.7. Application to Elliptic Variational Inequalities 874
32.8. Application to First-Order Evolution Equations 876
32.9. Application to Time- Periodic Solutions for Quasi- Linear
Parabolic Differential Equations 877
32.1 O. Application to Second-Order Evolution Equations 879
932.11. Regularization of Maximal Monotone Operators 88 t
32.12. Regularization of Pseudomonotone Operators 883
932.13. Local Boundedness of Monotone Mappings 884
932.14. Characterization of the Surjectivity of Maximal
Monotone Mappings 886
32.15. The Sum Theorem 888
32. J 6. Application to Elliptic Variational Inequalities 892
32.17. Application to Evolution Variational Inequalities 893
32.18. The Regularization Method for Nonuniquely Solvable
Operator Equations 894
32.19. Characterization of Linear Maximal Monotone Operators 897
32.20. Extension of Monotone Mappings 899
32.21. 3-Monotone Mappings and Their Generalizations 901
32.22. The Range of Sum Operators 906
932.23. Application to Hammerstein Equations 908
32.24. The Characterization of Nonexpansive Semigroups in H-Spaces 909
CHAPTER 33
Second-Order Evolution Equations and the Galerkin Method 919
33.1. The Original Problem 921
33.2. Equivalent Formulations of the Original Problem 921
33.3. The Existence Theorem 923
933.4. Proof of the Existence Theorem 924
933.5. Application to Quasi-Linear Hyperbolic Differential Equations 928
933.6. Strong Monotonicity, Systems of Conservation Laws, and
Quasi-Linear Symmetric Hyperbolic Systems 930
933.7. Three Important General Phenomena 934
33.8. The Formation of Shocks 935
33.9. Blowing-Up Effects 937
33.10. Blow-Up of Solutions for Semilinear Wave Equations 944
33.11. A Look at Generalized Viscosity Solutions of
Hamilton-Jacobi Equations 947
GENERAL THEORY OF DISCRETIZATION METHODS 959
CHAPTER 34
Inner Approximation Schemes, A-Proper Operators, and
the Galcrkin Method 963

Contents (Part II/B)
XIII
J4.1. Inner Approximation Schemes
34.2. The Main Theorem on Stable Discretization Methods with
Inner Approximation Schemes
34.3. Proof of the Main Theorem
*34.4. Inner Approximation Schemes in H-Spaccs and the Main
Theorem on Strongly Stable Operators
34.5. Inner Approximation Schemes in B-Spaces
934.6. Application to the Numerical Range of Nonlinear Operators
963
965
968
969
972
974
CHAPTER 35
External Approximation Schemes, A-Proper Operators. and
the Difference Method
35.1. External Approximation Schemes
35.2. Main Theorem on Stahle Discretization Methods with
External Approximation Schemes
35.3. Proof of the Main Theorem
35.4. Discrete Sobolev Spaces
35.5. Application to Difference Methods
35.6. Proof of Convergence
978
980
982
984
985
988
990
CHAPTER 36
Mapping Degree for A-Proper Operators
36.1. Definition of the Mapping Degree
36.2. Properties of the Mapping Degree
*36.3. The Antipodal Theorem for 14-Proper Operators
36.4. A General Existence Pnnciple
997
998
1000
1000
1001
Appendix
References
1009
1119
1163
1174
1179
1182
1183
1189
List of Symbols
List of Theorems
List of the Most Important Definitions
List of Schematic Overviews
List of Important Principles
Index

Contents (Part IliA}
Preface to Part II/A VII
CllAfiER..ll
Variational Problems. the Ritz Method. and
the Idea of Orthogonality 15

The Galerkin Method for Differential and Integral Equations,
the Iriedrichs Extension. and the Idea of Self-Adjointness 101
CHAnER..2O
Difference Methods and Stability 192
s
CHAfIElUl
Auxiliary Tools and the Convergence of the Galerkin
Method for Linear Operator Equations 229
CHAfiER..22
Hilbert Space Methods and Linear Elliptic Differential EQuations 314
CHAEIEJU1
l.filbert Space Methods and Linear Parabolic Differential Equations 402

Hilbert Space Methods and Linear Hyperbolic
Differential Equations 452
xv

GENERALIZATION TO
NONLINEAR STATIONARY
PROBLEMS
When the answers to a mathematical problem cannot be found, then the reason
is frequently the fact that Yle ha\'c not recognized the general idea, from which
the given problem appears only as a single link in a chain of related problems.
David Hilbert (1900)
In the preceding chapters we studied linear monotone problems. In the
follo\ving chapters we \\'ant to generalize these results to nonlinear monotone
problems.
(i) In Chapters 2S through 29 we investigate stationary problems, i.e., we study
operator equations of the form
Au = b,
ue X,
together with applications to quasi-linear elliptic differential equations and
to Hammerstcin integral equations.
In this connection we consider the following cases:
Lipschitz continuous, strongly monotone operators on H-spaces (Chap-
ter 25
monotone coercive operators on B-spaces (Chapter 26);
pscudomonotone operators (Chapter 27);
maximal monotone operators (Chapter 32).
For example, strongly continuous perturbations of monotone continuous
operators are pseudomonotone. In Part I \ve considered the two fundamental
fixed-point principles of Banach and Schauder. Figure 25.1 shows that these
two principles also playa crucial role in the theory of monotone operators.
469

470
Generalization to Nonlinear Stationary Problems
fixed-point theorem
of Banach
fixed-point theorem
of Brouwer
1
. fixed-point
theorem
of Schauder
existence principle
for equations in .Y
1
+ con'..crgence of the
Galcrkin method
via monotonicit), trick
1
main theorem on monotone
operators in B-spaccs
(Theorem 26.A)
+ contractivity
trick
main theorem on
Lipschitz continuous.
strongly monotone
operators in H-spaces
(Theorem 25.8)
Figure 25.1
The special case of variational methods will be considered in Chapter 25.
In this connection, the solutions of the convex minimum problem
f(u) = min!,
14 e X,
are solutions of the monotone operator equation
f'(14) = b,
ue X,
which generalizes the classical Euler equation.
(ii) In Chapters 30 through 33 we consider nonstationary problems, i.e., we
study evolution equations of first and second order
at") + Au = b,
n = 1, 2,
together with applications to quasi-linear parabolic and hyperbolic
equations.
In (i) and (ii), the operator A is monotone or, more generally. pseudo-
monotone. Here, the notion of maximal monotone operators plays a funda-
mental role.
(iii) In Chapters 34 through 36 we investigate a general theory of discretiza.
tion methods together with applications to Galerkin methods (inner
approximation schemes) and difference methods (external approximation
schemes).
In this connection, the notion of A-proper operators is crucial.

Basic Ideas of the Theory or Monotone Operators
471
Basic Ideas of the Theory of Monotone Operators
Riemann has sho\\'n us that proofs are better achieved through ideas than
through long calculations.
David Hilbert (1897)
One can understand a mathematical statement, if one
(i) can use it,
(ii) has completely understood the proof, or
(iii) one can independently find the proof again at any time.
Only when one reaches the third step can one speak of understanding in a real
sense.
Aleksander Ostrowski (1951)
Male lovers sometimes lack experience before their fortieth year. and later on,
there is often a lack of opportunity. In the same way. younger mathematicians
often lack the knowledge, and older ones lack the ideas.
Wilhelm Blaschke (1942)
The theory of nonlinear monotone operators is based on only a few tricks.
For the convenience of the reader, we summarize these tricks here. This way.
we want to make the proofs of the main results as transparent as possible. In
the following Chapters 26 through 36, the items (1 (2), etc. below, will be
quoted as (25.1), (25.2), etc.
The theory of nonlinear monotone operators generalizes the following
elementary result. We consider the real equation
(E)
f-(I') = b,
UE R,
and assume that:
(i) The function F: R -.. IR is monotone.
(ii) F is continuous.
(iii) F(u) -. + 00 as 14 -. :too,
Then, for each b E Rt equation (E) has a solution. If F is strictly monotone,
then the solution is unique (cr. Fig. 25,2).
This classical existence theorem follows from the intermediate value
theorem of Bolzano, whereas the uniqueness statement is obvious.
In particular, if f: R -. R is convex and C 1 t then F = I' is monotone, and
(E) with b = 0 is the Euler equation to the minimum problem
(M) f(u) = min!, U E R.
This observation is the key to the application of variational methods in the
theory of monotone operators. However, note that the general theory of
monotone operators concerns operator equations which are not necessarily
the Euler equations of extremal problems.

472
Gneralization to Nonlinear Stationary Problems
r
b - - - - -- - - - -
F
. U
Figure 25.2
We now want to generalize the result above to monotone operator equa-
tions of the form
(E*)
Suppose that:
(i*) The operator A: X ... X* is monotone on the real reflexive B-spacc X,
I.C.,
Au = b,
ue X.
(Au - Av, u - v)  0
(ii*) A is hemicontinuous, i.e., the map
I  (A(u + tv), ",.>
for all 14, v eX.
is continuous on [0, I] for all u, v, )\1 e X.
(iii*) A is coercive. i.e.,
I . (Au, u)
1m = +a;.
IIIIII-ex: lIu;t
Then the main theorem on monotone operators (Theorem 26.A) tells us the
following: For each b E X*. equation (E*) has a solution.
By Theorem 32.H, this fundamental result remains true if we replace (Hi.)
\vith the weaker condition that A is \veakly coercive, i.e.,
lim IIAuU = cc.
lIu, -. X)
If, in addition, A is strictly monotone, i.e.,
(Au - At" II - v) > 0 for all u, veX with II :I: v,
then the solution of (E*) is unique.
The result for equation (E) above is a special case of this theorem. In this
connection, set X = Rand
F(u) = Au.
Note that X. = Rand
(Au - Av, u - v) = (F(u) - F(v)(u - 1,')

Basic Ideas of tbe Theory of Monotone Operators
473
as well as
(Au, u)/llull = F(u)u/lul.
Conuently, the assumptions (i), (ii). (iii) above are special cases of (i*), (ij*),
(iii*), respectively.
In order to prove the main theorem on monotone operators for (E.) and
similar results, we use the Galerkin method. Here the existence proof proceeds
along the following lines.
Step 1: Solution of the Galcrkin equations.
In this connection we obtain equations in R". Let fl" be a solution of the
nth Galerkin equation.
Step 2: A prior; estimates.
We show that (u,,) is bounded.
Step 3: Weak convergence.
We sho\v that there is a subsequence (u".) with
u · -"'" u
.
as n  00.
Step 4: We show that u is a solution of the original equation Au = b, u E X.
We want to discuss this.
(1) Existence prillciples for the Galerkin eqllatiollS. In Step I we can use all
the existence results for operator equations considered in Part I. In particular.
we \vill use
(3) the existence principle for equations in R- (Section 2.4); and
(b) the antipodal theorem of Borsuk (Section 16.3).
(2) Coercit'eness and a priori estimates. Step 2 above is based on the follow-
ing result. Let A: X -. X* be a coercive operator on the real B-space X, i.e.,
(C)
(Au, 1I)/lIuli -. + X)
as lIu II -. 00.
Then, for fixed heX., the set of the solutions of equation Au = b, u E X, is
bounded.
Indeed, it follows from (C) that there is a sufficiently large R > 0 such that
( A U, II) > (1 + 211 b II) II u II ,
for all u e X with liull > R. This implies
(Au. u) - (b, II) > (1 + UbD)lIuli
 (1 + IIbll)R,
for all II e X with 111111 > R. Thus, Au = b implies 111111 < R.
(2a) N oncoercive problems al,d Fredholm allerl,atit'es. If the operator A:
X -+ X* is not coercive, then we need Fredholm alternatives for the equation

474
Generalization to Nonlinear Stationary Problems
Au = b, u e X, i.e., this equation has only solutions in the case where the
right-hand side b satisfies appropriate conditions. Such nonlinear Fredholm
alternatives \vill be considered in Chapter 29.
In Step 3 above "'e will use the following result.
(3) Main theorel1' on weak ('onvergence. Each bounded sequence (un) in a
reflexive B-space X has a weakly convergent subsequence (Theorem 21.D).
Step 4 above is based on the following trick which makes essential use of
the notion of monotone operators.
(4) Tile decis;t'e monotonic;ty Irick. Let A: X -t X. be a monotone and
hcmicontinuous operator on the rea) reflexive B-space X. Then:
(a) A is maxinJal monotone, i.e.,
(b - Av, u - v) > 0,
for all veX and fixed II e X, be X., implies Au = b.
(b) A satisfies condition (M). i.e., it follows from
UIIII in X as n... 00,
Au....... b in X.
as " -+ 00,
and (Au", II,,) -+ (b, u) as n -+ 00 or, more generally,
lim (Au., II,,) < (b,lI)
,,-oc
that Au = b.
(c) It follows from either
",,U in X
and
Au" -. b in X.
as II -. C().
or
II.. -+ u in X
and
Au b inX.
"
as n -+ ,
that Au = b.
PROOF. Ad(a). Let v = u - tw, where I > O. Then
(b - Av, u - v)  0
implies (b - A(u - 1\\'), ",)  O. The operator A is hemicontinuous, and
hence, letting t -+ 0, we obtain that
(h - Au, ",) > 0 for all ,,, e X.
Hence (b - Au, ",) = 0 for all w e X, i.e., b - Au = O.
Ad(b). (c). By the monotonicity of A,
(Au", II..) - (Av, u.) - (Au" - Av, v) = (Au" - Av, u" - v) > 0

Basic Ideas of (be ThCO!). of Monotone Operators
475
for all veX and all PI. Letting n  00, this implies
(b,u) - (Av,u) - (b - Av,v) > 0,
and hence
(b - Av, U - v) > 0
Cor all veX.
Since A is maximal monotone by (a we get Au = b.
(5) MonotoPlicit)' and uniqlleness.
(Sa) Operator eqllations. Let A: X -+ X. be strictly monotone on the real
B-space X. Then the equation
All = b,
ue X,
has at most one solution.
Indeed, if Ar4 = Av and u =1= v, then (All - Av, u - v) > 0 by the strict
monotonicity of A. This is a contradiction.
(5b) EvolutioPl equations. We consider the initial value problem
u'(I) + AU(I) = 0 for 0 < t < T,
(P)
11(0) = Uo.
Let "V c H s V." be an evolution triple. Suppose that A: V -+ V. is mono-
tone. Then, (P) has at most one solution u E W p 1 (0, T; J': H), where p is a fixed
number with I < p < 00.
To prove this, assume that u v e W p l (0, T; V, H) are solutions of (P). The
formula of integration by parts (Proposition 23.23) yields
! nu(t) - v(t)1I  - ! lIu(O) - v(O)II
= f (u'(s) - v'(s) u(s) - v(s) > ds
= - f (Au(s) - Av(s).u(s) - v(s»ds S 0
for almost all t E ]0, T[, since A is monotone. Noting u(O) = v(O) = uo, we get
lIu(t) - v(t)1I H S 0
for almost all t E ]0, T[.
Hence u(t) = vet) for almost aliI e ]0, T[, i.e., u = v in W p 1 (O, T; Jt: H).
(6) J\lonotonicily and contracl;v;ty. Let the operator A: X -+ X. be Lip-
schitz continuous and strongly monotone on the real H-space X, i.e..
(Au - Av, II - v) > enu - vll 2
for all u, veX and fixed c > O. Then the equation
(00)
Au = b,
UE X,

476
Generalization to Nonlinear Stationary Problems
is equivalent to the fixed-point problem
(6b) u = L,u. 14 EX.
\vhere L,u = u - IJ- 1 (Au - b) for fixed t > O. Here, J: X --+ X. is the duality
map of X. In Section 25.4, we will show by a simple computation that there
is at> 0 such that L,: X -. X is k-colJtracti'e. Thus, by the Banach fixed-point
theorem, we obtain a unique solution for (6b). Consequently, Cor each b EX.,
equation (6a) has a unique solution u. and the iterative method
U n "' l = L, UtI'
n = 0, 1, 2, . . .
converges to 14 as n -. w. for each given Uo eX.
(7) Monotonicity and cO"f;xit}.. Let f: X --+ R be a G-differentiable func-
tional on the real B-space X. In Section 25.5 we will show that:
f' X -. X. is monotone if)ef ;s convex.
Moreover, \\'e will show that if 1 is convex, then the minimum problem
f(u) = min!.
is equivalent to the operator equation
UE X,
f'(u) = O.
ue X.
Ho\vever, there are monotone operators A: X --+ X. which are not potential
operators. i.e.. A does not allow a representation of the form A = I'. In Section
41.3 we will show that an F -differentiable operator A: X --+ X. is a potential
operator iff the following symnJetr}' condition holds:
(A'(u)v, \v) = (A'(u)\v, v)
for all u, v, \\' e X.
If A is a potential operator. then the potential is given by
feu) = t' (A (tu), u) dt for all U E X.
In particular. let A: X  X. be linear and continuous on the real B-space
X. Then. A is a potential operator iff A is symmetric. i.e., (Av, ",) = (A""v)
for all v, \V EX. The potential is given by
l(r4) = t(Au,u) for all II e X.
(8) Weak seqlle"tiallo,,'er se,n;continu;t}' a,1d a general ,n;IJ;,nlln, principle.
Let M be a nonempty bounded closed convex set in the real reflexive B-space
X. Let f: J\f --+ fR be weakly sequentially lower semicontinuous, i.e.,
II" --'- 14 in JW
as n -+ OC)
implies
feu) < lim feu,,).
" ... fX\

Basic Ideas or the Theory or Monotone Operators 477
Then the minimum problem
(8a) f(ll) = min!, u e j\-I,
has a solution.
To prove this set
.2 = inf /(11).
-€M
By construction of , there is a sequence (u..) in I such that
lim f(u.) = .
a.x'
Since J'A is bounded, there exists a subsequence. again denoted by (u.. such
that
u u
n
as n  00.
Since M is convex and closed, we get u E M by Proposition 21.23(f). Hence
.(r4) < lim 1(11") = a,
"-«:
i.e., .(u) = a. Consequently, II is a solution of (8a).
In Section 25.5 we "'ill show that each continuous convex functional f:
X --. R is weakly sequentially lower semicontinuous.
(9) Tile ,nain theorenl 0" convex n,ininlum problenls. Let f: X  R be con-
tinuous and convex on the real reflexive B-space X. In addition. suppose that
f is weak Iy coercive, i.e.,
(9a)
lim /(u) = +CC.
11"1,  
Then the minimum problem
(9b)
f(ll) = min!,
UE X,
has a solution.
Indeed, it follo\\'s from (9a) that there is an R > 0 such that f(u) > f(O) for all
liE X -M, \vhere jW = {ueX: lIull R}. Consequently, it is sufficient to solve
the minimum problem (8a). Now, the assertion follows immediately from (8).
(10) TIle idea of regularization. In order to solve the equation
(lOa) Au=b, ueX,
we can consider the modified problem
(lOb) Au, + £Bu, = b, u( e X,
where E: > 0 is a small parameter. Sometimes it is easier to solve (lOb) than
(lOa). For example, it is possible that the operator A + £8 is coercive for £ > 0
and not coercive for £ = O. Now the idea of regularization is the following:
(i) We first solve the "regularized" problem (lOb) for all small £ > O.

478
Generalization to Nonlinear Stationary Problems
(ii) We show that the sequence (lie) of solutions of (lOb) is bounded in the
reflexive 8-space X for 0 < t S £0-
(iii) We choose a weakly convergent subsequence U e  u as t --+ 0 and show
that u is a solution of the original equation (lOa).
In Chapter 32 we shall present the theory of IJIQx;mal monotone operators,
\vhich forms the hard core of the theory of monotone operators. This theory
will be based on both the Galerkin method and the method of regularization
via duality map_
The following method of the Y osida approximation represents an impor-
tant regularization method for solving evolution equations.
(11) The trick of tlae Yosidtl approx inJal ion. We want to solve the initial
value problem:
(11 a)
lI'(I) + AlI(t) = 0,
ufO) = uo,
where u(t) lies in the real H-space X. Instead of (1Ia) we consider the regu-
larized problem:
u;(t) + A(l + tA)-1 "e(t) = 0,
o < t < .
(11 b)
o < r < 00,
UI:(O) = 1'0'
The operator A(l + eA)-1 is called the Y osida approximation of A. We assume
that:
(i) the operator A: D(A) s; X -. X is monotone; and
(ii) A is maximal accretive, i.e., for all t > 0, the operator (I + £A): D(A) --+ X
is bijective and the so-called resolvent
(1 + eA)-l: X ..... X
. .
IS nonexpanslve.
In Chapter 31 we will show that (ii) implies (i) and that the regularized
initial value problem (11 b) can be easily solved by using the Picard- Lindelof
theorem (Theorem 3.A), since the Y osida approximation is Lipschitz con-
tinuous. In order to solve the original problem (11 a), we have to investigate
the limiting process
Uc(t) ..... u(t) as t -. 0,
and we have to show that u(') is a solution of (11a). To this end, we win use
the convergence trick of maximal monotonicity (13) below combined \\'ith the
trick of maximal accretivity (12).
(12) The trick of maximal accretivilY. Suppose that A: D(A) 5 X  X is
monotone and maximal accretive on the real H-space X. Then A is maximal

Basic Ideas of the ThCOI)' of Monotone Operators
479
mono' one, i.e., if
(b - Avlu - v) > 0 for all v E D(A),
and fixed b, u e X, then u e D(A) and Au = b.
To show this, we choose v = v, where
v, = (I + A)-l(b + u + tz)
for fixed t > O. This implies v, e D(A). Adding
(b - Av,lu - v,) > 0 and (u - v,lu - v,)  0,
we get
(b + u - (1 + A)v,lu - v,) > o.
This is identical to
(zlu - v,) < o.
Letting t  0, we obtain that
(zlu - vo) = (zlu - (I + A)-l(b + u»  0
This implies u - (I + A)-I(b + u) = 0, and hence
u + Au = b + u,
for all z e X.
i.e.. Ar4 = b.
(13) Tire convergelJce trick of maximal monotonicity. Suppose that A:
D(A) e X.... X is maximal monotone on the real H-space X. Then
u" .... u and Au"  b as n .-. 00
implies Au = b.
The proof follows easily from
(Au" - Avlu n - v)  0
Let t i ng n .... 00, \ve get
(b - Avlu - v)  0
for all v e D(A) and all n.
for all v e D(A),
and hence Au = b, since A is maximal monotone.
This result is closely related to the monotonicity trick (4c) above.
In Chapter 31 we will use (11) through (13) above in order to construct
nonexpansive scmigroups on H-spaces. In Chapter 57, we will show that
maximal accretive operators also play an important role for solving multi-
valued evolution equations on B-spaces, and for constructing nonexpansive
scmigroups on B-spaces. In real H-spaces one has the following:
A ;s maximal accretiL'e iff A is maximal nwnotone.
(14) The convergence of approximation methods. Let A: X .... X. be an
operator on the real reOexive B-space X. We consider the equation
(E) Au = b, u eX.

480
Generalization to Nonlinear Stationary Problems
Suppose that (u..) is a sequence of approximate solutions in X, and suppose
that (u..) is bounded. By (3), there exists a \vcakly convergent sI4bsequellce of
(u,,). We want to answer the following two questions:
(i) When is the total sequence (u,,) weakly convergent to a solution of (E)?
(ii) When is the total sequence (u II ) strongly convergent to a solution of (E)?
(14a) Un;(/uelless and ,,'eak convergence of tile total sequence. Suppose that
the limit of an arbitrary weakly convergent subsequence of (II,,) is a solution
of (E), i.e.,
ulJ'  u
as n..... 00
implies All = b. Moreover, suppose that equation (E) has at most one solution.
Then, equation (E) has a unique solution u and
U II
II
as n.-. 00.
This follows immediately from the convergence principle in Section 10.5. The
same principle yields the following result.
(14b) U niql4elless and strong convergence of the tOlal sequence. Suppose that
each subsequence of (II,,) has, in turn, a convergent subsequence 14,.. -+ 14 as
n --. r:f.:,. and Au = b. Moreover, suppose that equation (E) has at most one
solution. Then equation (E) has a unique solution II, and
U. --. u
as n.-. ,x;,
(14c) CO'lverge'lce Irick for unifornll}' monotone operators. Let A: X  X.
be a uniformly monotone operator on the real reflexive B-space X. i.e., more
precisely, there is a c > 0 and a p > 1 such that
clill - lili P < (A14 - At'. u - v) for all u. I' e X.
Then the weak convergence
U..u in X
as n.-. 00.
together \vith Au..  Au in X. and <Au.., u,,) -+ (AI4, u) as n ..... <:1':-', implies the
strong convergence
u" ... u
as n.-. 00.
This follows from
c 1111 - u"II'  (Au - Au.., u - u,,)
= <Au, 14) - <Au, 14,.) - <Au.., u)
+ (Au.., u,,) -. 0 as n..... 00.
For the convenience of the reader we have used here a special case of the
general definition of uniformly monotone operators, which \vill be given in
(15a) below. It is easily shown that the result above also remains \'alid for
uniformly monotone operators in the general sense.
(14d) Densit) trick for \veak convergePJce. Let (u..) be a bounded sequence

Basic Ideas of the Theory or Monotone Operators
481
in the B-space X. Suppose that there are a dense set D in X. and an element
u in X such that
(f, un) -. (f, u)
as n-tOC)
for all fe D.
Then ""  u as n ..... 00 (cf. Proposition 21.23).
(14e) The Toeplitz trick and the co,uJergellce of projection--ileration metlwds.
Let (CI",) be a sequence of real numbers with a", -+ 0 as nl -+ 00, and let
o  k < I. Then, the classical theorem of Toeplitz tells us that
"
L k"-"'a m -+ 0
-=1
as II -. 00.
In Section 25.1, we will use this result in order to prove the convergence of
the general iterative method
U,,+1 = A"ll",
n = 0, 1, . . . .
In particular, if we set A. = PItA, where P" is a projection operator, then we
obtain the con\'crgence of the projection-iteration method corresponding to
the operator equation II = A,4.
(15) Stable operators. Let X and Y be real B-spaces. The operator A: X -+ y
is called stable iff
a(lIu - vII)  !tAu - Av!1
for all 14, VEX.
Here we assume that the function a: R.. -+ R. is strictly monotone increasing
and continuous \\'ith a(O) = 0 and
lim a(t) = +00.
, - ... ('.C.
For example, we may choose
a(t) = Cl P - 1 for all I  0,
and fixed c > 0, p > I.
(15a) U"ifor"II} monotone operators are stable. If A: X -+ X. is uniformly
monotone, i.e.,
a(lIu - vII) lIu - vII S (Au - Av, u - v)
then A is stable.
Indeed, for all u, veX, we get
a(Jj14 - vII) 1114 - vD  II Au - Avll Hu - vII.
for all u, v EX,
(ISb) Stable operators and uniqueness. Let A: X -+ Y be stable. Then, for
each beY. the equation
Au = b,
ue X,
has at most one solution.
To prove this. suppose that Au = Av. This implies a(lIu - vII) = 0, and
hence u = v.

482
Generalization to Nonlinear Stationar)' Problems
(ISc) Stable operarors and tile contilluous dependence of the solutions on tlte
right-hand side. Let A: X  Y be stable. Suppose that
Au" = b.. for all n and Au = b.
Then bIt  b in Y as II  00 implies Un --. II in X as " -. 00.
This follows from a(lIu" - ull) S II All" - Aull < lib.. - bll.
(15d) Stable operators and a priori estimates. Let A: X -+ Y be stable. Then,
for each beY: there is an R > 0 such that all the solutions of the equation
Au = b
satisfy the estimate lIuli s R.
This follows from a(lIulD s IIAu - A(O)II < IIbll + IIA(O)II and a(t) -. +00
as t  + .
(16) Tile .fundan,elltal properlJess trick and approxinJalion mell,ods. Suppose
that:
(H 1) The operator A: X -. Y is stable.
(H2) If (rl,,) is a bounded sequence in X with
All" -+ b
then there exists a subsequence
as '1 --. <X),
u". --. u
as n --. 00
such that Au = b.
Then it follows from
Au.. = bIt
and bIt  b as '1 -. XJ that the equation
Au = b,
for alllJ
UE X,
has a unique solution II, and u" -. u as n --. .
Indeed, the sequence (b,,) is bounded. From
a(lIunll)  IIAu.. - A(O)II S IIb,,1I + IIA(O)II
for all n
we obtain that (u ll ) is bounded. By (H2), there exists a subsequence rl,,' -+ u as
n .... 00 with Au = b. Since A is stable, the equation Au = b has at most one
solution. Now the assertion follows from (14b) above.
(16a) 7'lIe special case of proper nJaps. The map A: X  Y is called pro-
per jff the compactness of the set M implies the compactness of A -1 ("'I).
Obviously, assumption (H2) above is satisfied jf A: X ..... Y is proper and
continuous. This is why (16) is called the properness trick.
(16b) The fundanlental notion of A-proper maps. In Chapter 20 we have
shown that there is a close connection between stability, consistency, existence,
and the convergence of approximate methods. Furthermore, in Chapter 21,

Basic Ideas of Ihe Theory of Monotone Operators
483
we have shown that the operator equation Au = b, u e X. is approximation
solvable for important classes of linear operators A. In Chapters 34 and 3S
\ve will generalize these results to nonlinear operators in the framework of a
general theory of discretization methods. In this connection, the notion of
A-proper maps will playa fundamental role. The definition of A-proper maps,
with respect to inner and external approximation schemes in Chapter 34 and
35, respectively, will be based on a generalization of(H2) above, and the main
theorems in Chapters 34 and 3S will be modifications of the properness trick
(16), combined with the antipodal theorem of Borsuk.
(17) General strategies for solving nonlinear partial differelltial equations. To
prove the existence of solutions ofnonlincar partial differential equations, one
can use one of the following six basic methods:
(i) compactness and topological methods (Chapter 6);
(ii) variational methods (Chapter 2S
(iii) monotonicity, locally coercive (or semibounded) operators, and the
Galerkin method (e.g., Chapters 26, 27, 30, and 83);
(iv) refined iterative methods via interpolation inequalities (Chapters 21 and
83);
(v) semigroups (Chapters 19 and 31);
(vi) compensated compactness (Chapter 62).
The prototype for (i) is the Leray-Schauder principle. This principle tells
us that a priori estimates and compactness yield existence. In (ii) through (vi)
we do not need compactness. However, in (ii) and (iii) we use weak compact-
ness. The basic ideas of the modern method of compensated compactness will
be explained in Section 62.12. Furthermore, it is possible to use the theory
of A-proper maps from Chapters 34 and 35, in order to obtain constructive
existence proofs for nonlinear differential equations.
Summarizing, a modern tendency in the theory of nonlinear differential
equations is to weaken the compactness assumptions and to use refined
convergence arguments for approximation methods in order to obtain exis-
tence proofs.
In this connection, we also mention the imponant method of geometric
measure theory in the calculus of variations (cf. Giusti (1984, M» and the
method of the so-called variational convergence (e.g., G-convergence) offunc-
tionals and operators (cf. Attouch (1984, M».
(18) The modern strateg}' of using several spaces. To prove the convergence
of the iterative method or the Galerkin method, it is frequently important to
use more than one space. In this connection, we consider the following three
basic results in this volume:
(i) the refined Banach fixed-point theorem via interpolation trick (Theorem
21. H);

484
Generaljzation to Nonlinear Stationary Problems
(ii) the main theorem on locally coercive operators (Theorem 27.8);
(iii) the main theorem on semi bounded nonlinear evolution equations
(Theorem 30.8).
In (i), we use the fact that the iterative sequence (II,,) is bounded in the "very
nice" space X and k-contractive in the "poor" space Z. Then, using the
interpolation inequality, we obtain the convergence of (u II ) in the "nice" space
Y where
X !: Y  Z.
In terms of function spaces, the functions in X are smoother than those in Y
and, in turn, the functions in Y arc smoother than those in Z.
In (ii) and (iii)t we solve the Galerkin equations in the "nice" space Y: and
we prove the convergence of the Galcrkin sequence in the "poor" space Z
where Y s; z.
Recall that in Chapter 5 we have already encountered the hard implicit
function theorem (the Moser-Nash theorem), which is based on a modified
Newton method working in a family of B-spaces. This method is related to (i).
In Chapter 83, we will continue the study of the strategy (i) through (iii).
(19) Basic strategies for et'OIUlion equations. To solve evolution equations,
one can use one of the following methods:
(i) the Galerkin method (Chapter 30);
(ii) the method of linear semigroups for semilinear and quasi-lincarequations
(Chapter 19);
(Hi) the method of nonlinear semigroups (Chapter 31);
(iv) the method of timc-discretization (Chapters 57 and 83).
In connection with (ii) and (iii) we will use several reduction tricks to be
discussed in (20) through (22) below.
In (iv), the original equation
u'(t) + AIl(t) = f(t),
0<1<1:
11(0) = "0
is replaced by the following approximate problem:
u" - u.- 1 f
At + Au" = (nAt),
where U II is an approximation for U('It). Thu in each step n = I, 2, . .., one
has to solve the operator equation
n = I, l . . . ,
II" + t. Au" = t. f(nt) + 11"_1
for U lt . In this connection, the nJaximal accretit'eness of A plays a fundamental
role. Note that we use back\\'ard differences. This is important for proving the
convergence of this method.

Basic Ideas of the Theory of Monotone Operators
485
(20) The fixed-point trick for solving semilinear evolution equations. We
consider the semilinear initital value problem
(20a)
U'(I) + Lu(t) = g(II(I»).
1,(0) = 140'
where L is a linear operator, together with the following linear problem:
0<1<'1:
(20b)
U'(I) + LU(I) = f(t),
0<1<'1:
f'(O) = "0'
Let S be the solution operator to equation (20b) i.e.,
14 = Sf.
Then the original problem (20a) is reduced to the fixed-point problem
(2Oc) 14 = Sg(u).
For example, if we solve (2Oc) by means of the Banach fixed-point theorem,
then the iterative method
11,,+ I = 8g(u,,),
PI = 0, 1, 2, . . . ,
corresponds to solving the following family of initial value problems:
U"'l (t) + LUll + I (t) = g(II,,(t)), 0 < t < T,
U.+ 1 (0) = uo,
where PI = 0, 1, 2, . . . .
Instead of problem (20b). one frequently considers the integral formula
u(t) = e-rLuo + f e(s-r)L f(s)ds.
which yields a generalized (mild) solution of (20b). Here, e-,L corresponds to
the semigroup generated by L. This way the original semilinear problem (20a)
can be reduced to the nonlinear integral equation
(2Od) u(t) = e-'Luo + f e(s-I)Lg(u(s»ds.
The solutions of (20d) are called mild solutions of (20a). This method has been
used in Chapter 19.
(2 J) The fixed-point trick for .olving quasi-linear et'Olrition equations. We
consider the quasi-linear initial value problem
(21 a)
u'(t) + A(u(t»)u(t) = f(t),
u(O) = 140'
where the operator u...... A(v)u is linear for each fixed v. Suppose that, for each
0<1<'1:

486
Generalization (0 Nonlinear Stationary Problems
(21 b)
v. \ve are able to solve the corresponding linear problem
11'(1) + A (V(I»U(I) = 1(1), 0 < l < T,
u(O) = "0.
We set
" = Sv.
Then the original problem (2Ia) is reduced to the fixed-point problem
(21c) u = SUI
F"or example, if we sol\'e (21 c) by using the Banach fixed-point theorem, then
the iterative method
U,,+1 = SUn'
II = 0, 1, 2, · · · ,
corresponds to the following family of linear initial value problems:
11;+1 (I) + A (u,,(t»"I1+ I (t) = /(t), 0 < t < T,
"11.1(0) = Uo, n = 0, I.... I
(J)
(22) r",o reduction tricks for second-order evollllion equations. We consider
the nonlinear initial value problem:
U'(l) + AII'(t) + Bu(t) = /(t), 0 < t < T,
u(O) = UO, 11'(0) = U I'
(22a) Theftrst reduction trick. We set
,
V=II.
From (J) \ve then obtain the first-order system
U'(I) - vet) = 0, 0 < I < T,
v'(t) + Av(r) + Brl(t) = f(t),
with the initial condition u(O) = Uo, v(O) = U 1- Letting
W = (u,v)
and F = (0,/), we obtain the first-order equation:
""(1) + C\v(t) = F(I), 0 < t < 1:
w(O) = (uo, U 1)'
(22b) The second reduction trick. We set
(Sv)(t) = f v(s)ds + "0.
Letting U(I) = (Sv)(t), the original problem (J) is reduced to the first-order

Basic Ideas of the Theory of Monotone Operators
487
equation:
v'(t) + Av(t) + B(Sv)(t) = 1(1),
v(O) = U 1 ·
The first reduction trick has been used in Chapter 19. The second reduction
trick will be used in Chapters 33 and 56.
O<t<1':
(23) The fixed-po;'Jt trick for semilinear operator equations. Let L: X -. y
be a linear bijective operator. Then the semilincar operator equation
(23a)
Lu = g(u).
UE X.
Cc1n be reduced to the fixed-point equation
(23b) u = L -I g(u), u e X.
(24) Tile reduction oj- senJili"ear elliptic boundar}' value problems to abstract
Hammerstein eql,atiolJS. We consider the semilinear boundary value problem
- u(x) = g(u(x» on G,
(24a)
u = 0 on oG,
together with the linear problem
(24a*)
- L1u(x) = I(x) on G,
u = 0 on aGe
Let K be the solution operator to (24a*) in appropriate spaces, i.e.. the solution
u of (24a.) can be represented in the form
u = Kf.
This \A'ay. the original problem (24a) is reduced to the abstract Hammerstein
equation
(24b)
u = Kg(u).
Rccall that K is the abstract Green operator introduced in Chapter 22.
Equations (24a) and (24b) correspond to (23a) and (23b), respectively.
This method will be used in Chapter 28.
(25) Application to quasi-linear elliptic differential equations. Set Du =
(D 1 u... . ... D.u).. D i = C/ai" and
N
IDul 2 = L ID,uI 2 .
'=1
In Chapter 25, we study the variational problem
(25a) fG (qJ(IDuI 2 ) - 2fu)dx = min!, u e Wl(G),

488
Generalization to Nonlinear Stationar)' Problems
in (RH. The corresponding Euler equation reads as follows:
N
- L D,(cp'(IDuI 2 )D i u) = f on G,
1=1
(2Sb)
u = 0 on aG.
In the special case where q>(t) = t, we obtain the classical Dirichlet problem.
If the real function t t-+ q>(t 2 ) is convex, then (25a) (resp. (2Sb») corresponds to
a convex minimum problem (resp. a monotone operator equation) Au = b,
U E W 2 1 (G).
Problems of type (2Sb) describe nonlinear stationary conservation laws,
which appear frequently in mathematical physics.
(26) Monotone operators and quasi-linear elliptic equations. We set
."1
Lu = - L D.(F,(Du)
1=1
and consider the boundary value problem
Lu + F(u) = f on G,
(26a)
u = 0 on aGe
In contrast to (2Sb). this problem is not always the Euler equation to a
variational problem. In Chapters 26 and 27, we reduce (200) to the operator
equation
(26b) Au = b, u EX,
where X = Wpl(G), 1 < p < 00. Here G is a bounded region in RN, N  1, and
Du = (D1u,...,D.vu).
In order to guarantee that the operator A: X -+ X. is monotone. coercive,
and continuous, we need the following conditions:
(i) Monoton;city condition for the principal part Lu:
.'1
L (fi(D) - fi(D'»(D, - 0;)  0
i-I
for all D, D' e IR'''.
(ii) /vlonolonic;t}' condition for F(u):
(F(u) - F(v»(u - v)  0
for all u, v e R.
(iii) Coercivetless condition for Lu + F(u):
.'1 .'1
L fi(D)D i + F(u)u  c L I Dil' - b,
i=1 i-I
for all D E Rft., U E  and fixed c > 0, b  o.
(iv) Gro\vtIJ co,ulition: The functions f': IR --. Rand F i : lR. tI -+ R are continuous

Basic Ideas or the Theory or Monotone Operators
489
for all i, and there is a number d > 0 such that
IF(u)1  d(1 + lul,-I)
Ifi(D)1  d(l + IDI P - I )
for all u € A,
for all D e R N and all i.
For example, these conditions are satisfied for the generalized Laplace
operator
1\.
Lu = - L D i (ID.uI P - 2 DiU).
i-I
Let / E L 4 ( G) with p -1 + q -1 = I. As a special case of Proposition 26.12, it
follows that the boundary value problem (26a) has a generalized solution
u e X in the case where the assumptions (i) through (iv) above are satisfied.
(27) Pseudomonotone operators and quasi-linear elliptic differential equa-
1;0115. We consider again the boundary value problem (26a). But \\'e now
assume that the lower-order term F(u) does not satisfy the monotonicity
condition (ii) in (26) above. In this case, we can use the theory of pseudomono-
tone operators to be considered in Chapter 27. Then the operator A in (26b)
has the form
Au = Alu + A 2 u,
where A 1 and A 2 correspond to the principal part Lu and lower-order part
F(II), respectively. More precisely, we have the foUowing typical situation:
(a) the operator AI: X -. X* is monotone and continuous;
(b) A l : X -. X. is strongly continuous; and
(c) A = A I + A 2 is pseudomonotone and coercive.
The basic idea of the theory of pseudomonotone operators is that lower-order
terms generate strongly continuous perturbations of the monotone principal
part of quasi-linear elliptic differential operators.
Compared with the theory of monotone operators, the theory of pseudo-
monotone operators yields stronger existence results for quasi-linear elliptic
equations. For example, it follows from Proposition 27.9 that the existence
theorem for (26a) remains valid, if the assumption (ii) drops out in (26) above.
(28) Noncoercive problems and nonlinear Fredholm alternatives. As a typical
example, we consider the boundary value problem
- Au - pu + la(u) = f on G,
(28a)
u = 0 on oG,
where Jl is a given real parameter, and the function f: G .... R is given. We first
consider the corresponding linear problem
(28b)
-u - J.lU = f on G,
u = 0 on oG,

490
Generalization 10 Nonlinear Stationary Problems
(28c)
together with the eigenvalue problem
-u-pu=O on 0,
u = 0 on aGe
Roughly speaking, we have the following situation:
(i) Problem (2Se) has a sequence of eigenvalues (PII) with
o < PI S JJ2  · · .,
and /l"  + 00 as n -to 00.
(ii) If P is not an eigenvalue of(28c then problem (28b) has a unique solution
for each f.
(iii) If J.l is an eigenvalue of (2Sc), then problem (28b) has only solutions for
such f which satisfy a solvability condition.
Our goal is to translate these results to the nonlinear problem (28a). This
leads us to nonlinear Fredholm alternatives to be considered in Chapter 29.
In the nonlinear case we have the following situation:
(a) If JJ < PI and h(u)u  0 for all u e R, then (28a) corresponds to a coercive
problem of pseudomonotone type. Here we obtain a solution for each f.
(b) If JJ  PI' then the existence of solutions for (28a) essentially depends on
the structure of the nonlinear term II and on the relation between the range
of h: R -to IR and the set of eigenvalues {Pt ,P2'...}.
(c) In particular, in Chapter 29, we consider an interesting result due to
Landesman and Lazer (1969) where the ne cessa ry solvability condition
for (28a) is also sufficient.
There exists a very extensive literature on problems of type (28a) in the
critical case (b).
(29a)
(29) Hammerslein integral equations. The nonlinear integral equation
u(x) + fG k(x,y)f(u(y))dy = 0,
xe G
can be written in the form of the following operator equation:
(29b)
u + KFu = 0,
ue X,
which is called an abstract Hammerstein equation. Here we set X = Lz(G) as
well as
(Ku)(x) = fG k(x,y)u(y)dy
and (Fu)(x) = f(u(x». In Chapter 28, we will apply the theory of monotone
operators to equations (29a) and (29b). In this connection, we need the

Basic Ideas of the Thcol)t of Monotone Operators
491
monotonicity of the function f: R -+ R and the mono tonicity of K, i.e.,
(Ku,u) = f. k(x,}')u(x)u(}')dxdy  0 for all u e L 2 (G).
GxG
(30) The j"Jportallt role of growth conditions in the theor}' of non/i,zeaT
dijJerential and integral equations. One of the typical difficulties in the theory
of nonlinear differential and integral equations is based on the following fact:
(A) The Lebesgue space L 2 (G) is not a Banach algebra. i.e., u, v e L 2 (G) does
IIot imply uv e L 2 (G).
For example, set G = ] -1, I[ and consider the functions
u(x) = v(x) = Ixl- 1 {3.
Then u, v e Lz(G), but rlv, L 2 (G).
In order to overcome this difficulty, one needs growth conditions. To
explain this, consider the continuous function f: R ... R, and suppose that
there is a e > 0 such that the following growth condition holds:
(308) If(u)1  e(l + lul) for aU u e R.
Let G be a bounded region in R N , N > 1. Set
(Fu)(x) = [(ll(X») for all x e G.
Then
II e L 2 (G) implies Fu e L 2 (G),
i.e., F is an operator from L 2 (G) to Lz(G). Indeed, if u e L 2 (G), then
fG I(Fu)(x)1 2 dx S 2c 2 fG (1 + lu(xW)dx < 00.
The foUowing observation is crucial. In order to relax the growth condi-
tion (30a we can use the Sobolev embedding theorems A 2 (45). To explain
this, suppose that, in contrast to (30a), we have the less restrictive growth
condition
(30b) If(u)J s; e(1 + lul 2 ) for all u e R.
Let G be a bounded region in 1R3. Then,
u e W 2 1 (G) implies Fu e L 2 (G),
i.c., F is an operator from W Z I(G) to L 2 (G). Ind the embedding
W 2 1(G)  L 4 (G)
is continuous, i.e., there is ad> 0 such that
(30e) JJuU 4 < d II uil i . 2 for all U E J(tZI (G).

492
Gcncrali7.8tion to Nonlinear Stationary Problems
If U E W 2 1 (G), then u e L 4 (G) and hence
fG I(Fu)(x)1 2 dx  2c 2 fG (1 + lu(x)I.)dx < 00.
In order to investigate the properties of nonlinear differential and integral
operators, one can use the following tools:
(i) growth conditions:
(ii) the Holder inequality;
(iii) the Sobolev embedding theorems; and
(iv) the fundamental Gagliardo- Nirenberg interpolation inequalities.
Typical examples for (iv) have been considered in Section 21.23. To explain
(ii) and (iii), let us consider the integral
J(Il) = fG,u2Diu,dx Corall ueW 2 1 (G),
where G is a bounded region in R 3 . We want to show that J(u) < OCt Indeed,
the Holder inequality for three factors yields
fG luuD,uldx < (fG u 4 dx Y.14 (fG u 4 dx YI4 (fa (D,U)2dx Y IZ
S lIulllIulll.2'
Using the Sobolev embedding theorem (30e), we obtain that
)(14) S d 2 Ur4 iI:. 2 for all u e "-'2 1 (G).
Observe the following:
(!X) In order to relax growth conditions, one can use Orlicz spaces. This will
be studied in Chapter 53.
(fJ) In the case of spaces of smooth functions (e.g., the space C( G ) or C m . 2 (G»),
we do not need any growth conditions.
However, since the spaces in (/1) are not reflexive, we cannot apply typical
results of the theory of monotone operators to this situation. In Part I we used
(fJ) in order to obtain existence results for nonlinear differential and integral
equations via fixed-point theory.
(31) The difference bet,veen K-monotone and monotone operators. Let
f: iii -+ R be a monotone increasing function, i.e.,
(3Ia)
U < v
implies
f(u) < f(v
and this is equivalent to
(31 b) (f(u) - f(v)(u - v) > 0 for all u, v E R.
Let X be a real B-space. Then, (31a) and (31 b) lead to the following two

Basic Ideas of the Theory of tonotonc Operators
493
different generalizations:
(i) K-monotone operators. We first generalize (31a). Let K be an order cone
in X. As in Chapter 7, we write u  v iff v - u e K. Then the operator
F: X -. X is called K-monotone or also monotone increasing iff
u  v implies F(u)  F(v).
(ii) MOIJolone operators. We now generalize (3 I b). The operator F: X -. X.
is called monotone iff
(F(u) - F(v),u - v) > 0
for all
u, veX.
The following two examples should illustrate these two different concepts.
Let f: R -. R be a continuous monotone increasing function. Set
(Fu)(x) = f(u(x».
EXAMPLE 1 (K-Monotone Operator). Let X = C[a,b] with -00 < a < b < 00.
and let K = C + [a, b]. i.e., X consists of all continuous functions u: [a, b] ..... 
and K consists of all nonnegative functions in X. Then the operator
F: X -. X
is K-monotone. Indeed, for all x e [a,b],
fleX) s vex) implies f(u(x») s f(v(x).
EXAPtIPLE 2 (Monotone Operator). Let Y = L;z(a, b) with -00 < a < b < 00,
and suppose that there is a c > 0 such that
1/(11)1 < e(1 + lul)
for all u e R.
Then the operator
F: Y -. Y.
is monotone. Indeed, for all u.. v e L 2 (a, b), we obtain that
(Fu - Fv,u - v) = I b (f(u(x) - f(v(x))(u(x) - v(x))dx > O.
Roughly speaking, we have the following typical situation:
(a) The theory of K-monotone operators can be applied to semilinear elliptic
equations of second order by using the maximum principle (cr. Chapter 7).
({J) The theory of monotone operators can be applied to quasi-linear elliptic
equations of order 2m with m = I, 2, .... In contrast to «X we need
restrictive growth conditions.
Some relations between K-monotone and monotone operators are con-
sidered in Glashoff and Werner (1979).

CHAPTER 25
Lipschitz Continuous, Strongly
Monotone Operators, the
Projection-Iteration Method, and
Monotone Potential Operators
For any continuous function f: R  LQ gro\\'ing everywhere strictly faster than
the function g(x) = x. the equation
x = f(x)
has a unique solution. This solution can be obtained by the iteration method
X""1 = (I - , ) x" + tf( x.), n = 0. 1, 2. . . . ,
where t is a sufficient)' small positive number.
The purpose of this note is to show that the above theorem, and its cor-
responding local form , remain valid in Hilbert spaces, provided the notion of a
function growing faster than another is adequately extended.
A fundamental tool in our argument is the Banach fixed-point theorem.
Eduardo Zarantonello (1960)
In this chapter we want to study the following topics.
(i) We first consider the operator equation
Au = b, U E X,
(32)
where the operator A: X -+ X. is strongly monotone and Lipschitz con-
tinuous on the real H-space X. We prove that, for each b E X., there exists a
unique solution of (32). The idea of proof is to apply the Banach fixed-point
theorem to the equivalent operator equation
u = u - l}-I(A" - b),
u e X,
(32a)
where t > 0 is an appropriate parameter, and J: X -+ X. is the duality map
of X. We also prove the convergence of the iterative method
II" = U"-l - tJ- 1 (Au a _ 1 - b).
n = 1, 2. . . . ,
(32b)
495

496
25. Lihitz Continuous. Strongly Monotone Operators
and the convergence of the important projection-iteration metlwd
u" = u lI - 1 - tP II J- 1 (AII"_1 - b), u" E XII' n = I, 2,..., (32c)
where Uo = 0, and XIS X 2 S ... £ X \vith X = U" Xn. Here, PIt: X ..... XII is
the orthogonal projection operator from X onto the fioite-dimensionallinear
subspace X n of X.
(ii) We solve the minimum problem
f(u) = min!,
together with the Euler equation
f'(u) = 0,
ue X,
II e X,
where X is a real reflexive B-space. In this connection, we shall prove the
following fundamental results:
the main theorem on minimum problems;
the main theorem on convex minimum problems;
the main theorem on monotone potential operators;
the main theorem on pseudomonotone potential operators.
(iii) As an important application of the abstract results to differential equa-
tions, \ve consider the conservation law
Lu = f on G,
II = 0 on oG,
(33)
\\'here Du = (D 1 u, . . . , DNu), and
.'i
LII = - L D i ("'(IDuI 2 )D,u).
1=1
Such problems occur frequently in mathematical physics (e.g., in hydro-
dynamics, plasticity, rheology, thermodynamics, electrodynamics, etc.). Prob-
lem (33) is the Euler equation to the minimum problem
fG (cp(IDuI 2 ) - 2fu)dx = min!,
II = 0 on aG.
Here, '" = cp'. The function cp depends on the constitutive law of the material.
Depending on the qualitative behavior of the function tp, we shall prove the
following results for (33).
(a) Existence and uniqueness of solutions. In this conncction problem (33) is
reduced to an operator equation of the form (32) with X = W 2 1 (G). The
equivalent operator equation (32a) corresponds to the modified boundary

25.1. Sequences of k-Contractive Operators
497
value problem
-du = -l1u - t(Lu - f) on G,
" = 0 on oG,
(33a)
\vhere t > O.
(b) Convergence of the iterative method
-l1u" = -I1U"-1 - t(Lll"_1 - f) on G t
u" = 0 on oG,
n = 1, 2. . . . , Uo = O.
(33b)
This corresponds to (32b).
(c) Convergence of the projection-iteration method.
(d) Convergence of the Ritz method.
(e) Duality and two-sided a posteriori error estimates for the Ritz method.
(f) Convergence of the Kacanov iterative method
N
- L Di((IDu"-112)Difl,,) = f on G,
i=1
". = 0 on cG,
n = 1, 2, . . . ,
(34)
where U o = O. This is a linear boundary value problem for U II .
The projection-iteration method represents a modification of the iterative
method (33b). In contrast to (33b we only approximately compute the
function ".. by using a Ritz method with n basis functions.
The decisive advantage of the projection-iteration method for nonlinear
problems is that, in each step. we have only to solve a finite linear system of
real equations.
25.1. Sequences of k-Contractive Operators
We consider the convergence of the iterative method
- T. aI(,,)
U.. - . ",,-I'
n = 1 t 2, . . . ,
(35)
for given "0 e M, where m(n) e N. In the special case T,,1II(n) = T, we obtain the
iterative method u.. = TU"_I corresponding to the Banach fixed-point theorem
in Section 1.1. We make the following assumptions.
(HI) Let M be a nonempty complete metric space with metric d.
(H2) For each n e Nt the operator T,,: M .... M satisfies the contractivity
condition
d(T,.u, T,.v)  k"d(u.. v),
for all u, l' E J\f and fixed kIt e [0, 1 [.
(36)

498
25. Lipschitz Continuou Strongly Monotone Operators
(H3) By the Banach fixed-point theorem (Theorem I.A), the equation
VII = T,. VII'
VII EM,
(37)
has a unique solution for each n e N. Suppose that (VII) converges to
u e JW, i.e..
d(vlI' u) -+ 0
as n --+ 00.
Proposition 25.1. Assume (H I) through (H3). Then the iterative sequence (un)
constructed in (35) converges to u in tile case \vhere one of the lollo,,';ng ''''0
conditions is satisfied.
(i) m(n) = 1 for all n e N, and SUPIIC  k < I.
(ii) m(n) > c/( I - k,,) for all n e Nand fi"y,ed c > O. and k n --. 1 as n --t 00.
This result \\'ill be used in the next section in order to prove the convergence
of the projection-iteration method.
PROOF. We will use the classical convergence theorem of Toeplitz, which we
recall in Problem 25.1.
Ad(i). Let k = sup k. For n = 2, 3, ..., we get
d(uII.v II ) = d(T,.lI n - l . T,.v lt ) S kd(u"_I' vn)
 k[d(u-1,V"-1) + d(v n -. , v,,)].
Repeated application of this inequality yields the key estimate
11-1
d(uII,v II ) S; k"- 1 d(1I 1 , VI) + L k"-'" d(v"" v"'+ I).
-=1
(38)
We set a",=d(v.,.,v..+ 1 ). By (H3), a..-'O as m--+oo. Since O < k< I, the
Toeplitz convergence theorem tells us that
,,-I
L k"-"a.. -. 0
'" IiiI I
as n -. 00.
Hence d(u lI , v) --. 0 as n -. 00. By (H3),
d(u, 14,.) < d(u. VII) + d(v,., u II ) -. 0
as n  00.
Thus, Un -. fl as n --+ 00.
Ad (ii). \Ve reduce this case to (i). To this end, we set All = 1;.,"(11). By (H2),
d(Allu, A"v)  k:(II)d(u, v) for all fl, v e J\1.
By (H3), Anv,. = V n for all n. Since kll -. I as n --+ :X), we obtain
k:,clI)  (1 - (I - kll)Y'(J-t"J --. e- c as n -. 00,
and hence sup."o k:(II) < 1 for sufficiently large no. We now apply case (i) to
the equation
Un = Anu,,-I'
n = no, no + I,...
o

25.2. The Projection-Iteration tethod for k-Contractive Operators
499
25.2. The Projection-Iteration Method for
k-Contractive Operators
We consider the equation
u = Bu,
ue X,
(39)
and make the following assumptions.
(A 1) The operator B: X ... X is k-contractive on the real separable H-space
X, i.e., there is a k e [O 1 [ such that
IIBu - Bvll  kllu - vII
for all u, veX.
(A2) (X") is a Galerkin scheme in X, where X n = span {''1".. ., 'Va'''}.
(A3) P,.: X .... X" is the orthogonal projection operator from X onto Xn.
We formulale the following three approximation methods.
(i) Iteralion method. For given Uo E X and n = I, 2, ..., let
u" = BU,,_I.
(40)
(ii) ProjecliolJ method (Galerkin method). For dim X = 00 and n = I, 2, ...,
let
v. = P.Bv",
v" EX".
(41)
Since Pn is self-adjoint equation (41) is equivalent to the Galerkin
equations
( v" I 'Vi..) = (Bv.. I 'Vt,,), k = 1, · . · , n',
where v" = Lt1 Ctll "'b. Here, the real coefficients C i .. are unknown.
(iii) Projection-iteration method. For dim X = 00 and n = I, 2, ..., let
""" = PnB'v.- I ,
, eX",
(42)
where Wo = O. Equation (42) is equivalent to
("',,I ""ill) = (Bw..-11 "'t..), k = I,..., n',
where,v n = L;I d lc ,,"'''. Thus, we obtain a linear system for the unknown
real coefficients db of "'..
Theorem 25.A. Assume (AI) through (A3). T/ren:
(a) The original equation (39) lias a unique solution u.
(b) The ileration nlethod (40) converges, i.e., u" -+ u as n -+ 00, and ,ve have the
error estimates
1111 - u"il  k-(I - k)-11111 1 - uoll for n = 1, 2 ... .
(c) The projectioll method (41) converges, i.e., for each n, equation (41) lias a
unique solution v"' and v" -+ U a. n -+ 00. Moreover, "'e have the e"or

500
25. Lipschitz Continuo Strongly Monotone ()pcrators
estinJaleS
lIu - villi < (I - k)-I dist(u, X,,)
,for n = 1, 2, . .. .
(d) The projection iteration nJethod converges, i.e., "'n --. fl as n .... 00.
PROOF. Ad(a). (b). Cf. Theorem I.A.
Ad(c). From Ii p.1I = I it follows that
IIP"Bu - PnBvll < IIBI4 - Bvil  kllu - ('11
for all  v EX,
i.e., P"B: X -. X is k-contractivc. According to the Banach fixed-point theo-
rem (Theorem I.A), equation (41) has a unique solution V n , i.e.. tIn = PIIBvft' Let
Bu = u. From
II P"BPnu - P"Bvnll  k IIP"u - v,,11
and the Schwarz inequality l(ulv)1 S rlullllvil. we obtain the ke}' estimate:
(I - k)lIv n - p.uU 2 < (v" - Pnulv" - P"Il) + (P.BPIlIl - P"Bvftlv. - P"Il)
= (P"BP"u - P"Bulvft - Pllu) S kllP"u - ullllv n - p"ull.
Hence
IIv n - P"u:1 S k(1 - k)-lllp.u - ull.
This implies
II Vft - 1111 S II v" - Pftull + IIP"u - ull  (I - k)-lll P"u - ull.
Since P"fl -+ fl as n  oc., we obtain v"  u as n  00.
Ad(d). We set T,. = P"B. Since I1P,,!J = I, we obtain
l11;.u - 1;.vll < II Bfl - BvlI < klill - vII,
for all u, t' E X. Now, the assertion follows from Proposition 25.1 (i). 0
25.3. Monotone Operators
The following definitions are basic.
Definition 25.2. Let X and Y be real B-spaces, and let A: X -. X. be an
operator. Then:
(i) A is called monotone iff
<Au - Av, u - v)  0
(ii) A is called strictly nJOIJotone iff
for all u, VEX.
(Au - Av. u - v) > 0
for all u, veX with u #= v.
(iii) A is called strongly nJonolone iff there is a c > 0 such that
(Au - Av, fl - v)  ellu - vll 2 for all 14, veX.

25.3. Monotone Operators
501
(iv) A is called ullifOrnll} monotone iff
(Au - Av, u - v)  a(lIu - vll)lIu - vII
for all II, I' e X,
where the continuous function a: R+ -. R... is strictly monotone increas-
ing with a(O) = 0 and aCt) -. +00 as t -. +00.
For example, we may choose a(t) = Clll,-1 with p > I and c > O. In
this case. we obtain
(Au - Av, II - v) > ellu - vll P
(v) A is called coercive iff
for all u, veX.
1 - (Au, 14 >
1m = +00.
IUII-r7) lIuli
(vi) A is called ,,'eakl}' coerC;t'e iff
lim UAull = 00.
HIIII'" :(1
(vii) The operator A: X ..... Y is called stable iff
nAu - AvU  a(lIu - vII) for all u, v E X,
where the function a(. ) is the same as in (iv) above.
(viii) The operator A: X -+ X on the H-space X is called strongly stable iff
there is a c > 0 such that
I(AII - Avlu - 1,>1 > ellu - vll l for all u, v € x.
By the Schwarz inequality, this implies HAu - Avll > ellu - vII for all u.
t' E X.
Obviously, we have the following implications:
A is strongly monotone  A is uniformly monotone =>
=> A is strictly monotone => A is monotone. (43)
Furthermore. we obtain:
A is uniformly monotone  A is coercive and stable. (44)
This follows from
(Au, u) = (Au - A (0), u) + (A (0), u)
> a( lIuli )fllIf) - IIA(O)lIlIuli
and
IIAII - Avllilu - vII > (Au - Av, U - v)  a( lIu - vII )lIu - vU.
Hence, if A: X -. X. is uniformly monotone, then
II Au - Avll  a( IJr4 - vII)
for all u, VEX.

502
25. Lipschitz Continuous. Strongly Monotone Operators
EXAt.tPLE 25.3 (Linear Monotone Operators). Let A: X -+ X. be a linear
operator on the real B-space X. Then:
(a) A is monotone iff A is positive, i.e. t (Ar4,u) > 0 for all U E X.
(b) A is strictly monotone iff A is strictly positive, i.e., (Au, u) > 0 for all
14 e X with u  o.
(c) A is strongly monotone iff A is strongly positive, i.e., (AI4 t U)  cllull 2
for all u E X and fixed c > o.
PROOF. Note that Au - Av = A(u - v).
o
EXAMPLE 25.4 (Monotone Real Functions). We consider the function f: R  R.
We regard f as an operator from X to X. \\rith X = R. Then,
(f(u) - f(v), 14 - v) = (/(14) - f(v»(u - v) for all II. v e Di.
This yields the following results.
(a) f: X -+ X. is (strictly) monotone iff f: R -+ R is (strictly) monotone
. .
IncreasIng.
(b) f: X -+ X. is strongly monotone iff
· f f(u) - f(v) 0
In > .
utl-,. U - V
(c) f: X ..... X. is coercive iff
lim f(u) = :too.
u
(d) If F: Oi -+ R is C 2 satisfying
F"'(u)  c for all u e R and fixed c > Ot
then
(F'(u) - F'(v»(u - v)  C(II - V)2
i.e., F': R -+  is strongly monotone.
(e) If F: R -+ R is C 1 satisfying
F'(u) - F'(v)  c(u - v)t
for all u, v e 
for all u, v e R with u > v and fixed c > 0, then F': R .... R is strongly
monotone.
EXAMPLE 25.5. We consider the function g: IR .... R defined by
g(u) = { IU 1P - 2 u f U:F 0,
o If u = o.
Then:
(a) If p > 1, then 9 is strictly monotone.
(b) If p = 2, then 9 is strongly monotone.
(c) If p  2, then 9 is uniformly monotone.

25.4. The Main Theorem on Strongl)' Monotone Operators
503
PROOF. This follows immediately from the inequality
(luIP-1u - Ivl,-2 v )(u - v)  clu - vi',
for all '4, v E (Ii and fixed p > 2, c > o.
To prove (45) we first consider the case 0 S v S u. Then,
f. U-
U,,-I - V,,-I = 0 (p - 1 Ht + V),,-2 dl
> f:- v (p - 1)1,,-2 dt = (u - V),,-I.
In the case v S 0  u, we use the inequality A 2 (30b) in order to obtain that
14 p -1 + I v I P - 1 > C (14 + II'I)P -1 . ( 46)
o
(45)
Proposition 25.6. Let A: X -. X* be an operator on ,he real B-space X. We set
/(1) = (A(u + tv), v) for all t e R.
Then the lollo,,'ing t\VO stalemenlS are equivalent.
(a) Tile operator A is monotolle.
(b) Tile functioll f: [0, 1] -. R is monotone increasing for all u, VEX.
PROOF. If A is monotone, then for 0 < s < t,
/(t) - f(s) = (I - S)-I (A(u + tv) - A(u + sv), (t - s)v)  O.
Conversely, if f: [0, 1] -. R is monotone increasing, then for 14, veX.
(A(u + v) - Au, v) = 1(1) - 1(0)  o.
o
25.4. The Main Theorem on Strongly Monotone
Operators, and the Projection-Iteration Method
We consider the operator equation
Au = b,
U E X"
(47)
and make the following assumptions.
(HI) The operator A: X -. X. is strongly monotone and Lipschitz con-
tinuous on the real H-space X, i.e., there are numbers c > 0 and L > 0
such that, for all u, v E X,
(Au - Av,u - v) > cllu - vll 2
and
IIAu - Avll < Lllu - vII.

504
25. Lipschitz Continuous. Strongly Monotone Operators
(H2) Let dim X = , and let (XII) be a Galcrkin scheme in the separable
H-space X, where X. = span {Will'...''''",,,}. Moreover, let PIt: X --+ X.
be the orthogonal projection operator from X onto XII'
The idea of our existence proof is to replace (47) by the equivalent operator
equation
u = Bll,
ue X,
(48)
where
Bu = u - tJ- 1 (Au - b).
for fixed, > O. Here. J: X .... X. is the duality map of X. We shall show that,
for 0 < t < 2c/L 2 , the operator B: X -+ X is k-contractive with
k 2 = 1 - 2ct + ,2 L 2 < I.
Therefore, \ve can apply Theorem 2S.A to equation (48). In particular, we can
solve equation (48) by means of the following approximation methods.
(i) Iteration Inethod. For given Uo e X and n = 1, 2, ..., let
U" = U._ I - tJ- 1 (Au"_1 - b).
(49a)
(ii) Projection nlethod (Galerkill nlethod). For II = I, 2, ..., let
VII = p.(v" - tJ- I (Av. - b»). v" EX",
.
I.C.,
P"J- 1 (Av II - b) = 0,
V. E XII'
(49 b)
(Hi) ProjectiolJ-;teratioll ,,,ethod. For \Vo = 0 and II = I, 2, . . ., let
\v. = P,,(\"'''_I - tJ- 1 (A\v._ 1 - b) "'" e XII' (49c)
This equation is equivalent to
( \"',.1 "'kn) = ("'" -I I "'b) - t ( A \"'" - 1 - b, \\tll >,
wherc k = 1, ..., n' and
,,'
"'ft = L d tit \"'tll
k"l
with the unknown real coefficients d tll of "'". In this connection, note that
(J- 1 bl\\.') = (b, ",) for all b e X*, w e X.
The follo\ving theorem shows that the original problem (47) is well-posed
and uniquely approximation-solvable.
Theorem 25.8 (Zarantonello (1960»). Assume (H I). Then, for each bE X*. the
operator equation Au = b, u e X, has a uniqrle solution.
The solution u depellds continuously Oil b. More precisely, ;1 10110"'5 from
Au) = bJ,j = I, 2. that
lIu 1 - u21 :5; c-'llb s - b 2 11,

25.4. The Main Theorem on Strongly Monotone Operators
505
alJd ,he inverse operator A-I: X. -. X ;s Lipschitz contiIlIlOIl." with Lipschitz
constalJt c- I .
Corollary 25.7. Assume (HI) and (H2). Choose a fixed t \vith 0 < t < 2c/L 2 .
Theil, as n -. 00, the approximation methods (49a) through (49c) contV!rge to the
unique solutioll of the eqllat;oll Au = h, " E X.
In particular. for each n E N, tile Galerkin equation (49b) has a rilliqlle ."olutioll
t'II' and K'e get the error estimate
(E)
IIv" - ull < c- 1 Ldist(rl, Xn
\vhere dist(lI, X n ) -. 0 as n -. XJ.
Similarly as in Section 22.22e, relation (E) is very useful for the finite clement
method. In fac (E) allows us to estimate the rapidity of convergence of the
finite element method.
PROOF. The case X = {O} is trivial. Assume that X :F- {OJ. Set C = )-1 A. For
all II, r; EX, we obtain that
(CII - Cvlu - v) = (Au - Av, U - v) > cflu - v1l 2 (50)
and
HCu - Cvll  IIAu - Avll s Lllu - vII,
by (HI). Note that 11)-111 = 1. This implies the ke}' inequality:
IIBu - Bvll 2 = Hu - vll 2 - 2t(CII - Cvlu - v) + t 2 11Cu - Cvll 2
 k 2 11u - vll 2 for all II, VEX,
where
k 2 = 1 - 2tc + t 2 L2.
By (50), IICII - Cvll  crlu - vJ! for all u, VEX. Hence 0 < c S L. Now, an
elementary discussion shows that 0 S k < 1 if 0 < t < 2c/ L 2 .
Consequently, the operator B: X --+ X is k-contractive. By the Banach
fixed-point theorem (Theorem I.A the equation u = Bu, u e X. has a unique
solution. Hence the equivalent equation Au = h, II e X, also has a unique
solution.
Now let Au) = b). Then
cllu. - 142112 S (Au. - AUz,u l - "2)
S IIAu, - AU21111u1 - u 2 11.
and hence :1111 - 11211  c-1rtAU I - Auzll. This implies IIA-lb! - A- I b 2 11 
c- 1 11b 1 - b 2 11 for all h., b 2 e X.. The proof of Theorem 25.8 is complete.
Corollary 25.7 follows from Theorem 2S.A. In order to prove (E), we first
note that the Galcrkin equation (49b) is equivalent to
()-l(Av" - b)lv) = 0 for all ve Xn.

S06
25. Lipschitz Continuous. Strongly Monotone Operators
By (21.30). this is equivalent to the equation
(Avft - b,v) = 0 for all v E X ft ,
\vhere we are looking for v" e X.. This implies the key relation
(Avft - b, v -- v,,) = 0 for all I' e XII.
Noting that Au = b, we get
(Av" - Au, v" - u) + (Av. - All, u - v) = 0,
for all v E X". Hence
cl1v" - ull 2 S (Av. - Au, v" - u)
S IIAv" - Auliliu - vII
 L"v" - uliliu - vII,
for all v e Xn. This implies (E).
o
25.5. Monotone and Pseudomonotone Operators,
and the Calculus of Variations
In the following it is our goal to study the important connection between
variational problems and the theory of monotone operators.
In the preceding section we considered the monotone operator equation
Au = b,
II e x.
(51)
We now investigate the special case that there exists a functional f: X -. R
with /'(u) = Au - b on X, i.e., we consider the operator equation
f'(u) = O. u e X, (52)
together with the corresponding minimum problem
I(u) = mint, u eX. (53)
An operator B: X .... X. on the B-spacc X is called a potential operator iff
there exists a G-differentiable functional f: X --. IR such that B = f'. Our plan
is the following:
(i) We discuss the connection between the convexity of f and the mono-
tonicity of f'.
(ii) We show that the solutions or(S3) are also solutions of (52). This way, we
obtain an important method in order to solve operator equations of the
form (52) by solving minimum problems. Equation (52) is the abstract
Euler equation to the variational problem (53).

25.S. Monotone Operators and the Calculus of Variations
507
(iii) We prove existence theorems for convex minimum problems and, more
generally, for minimum problems with weakly sequentially lower semi-
continuous functionals.
(iv) This leads very simply to existence theorems for monotone and pseudo-
monotone potential operators (Theorems 25.F and 2S.G).
(v) In the following two chapters we shall show that the existence theorems
in (iv) can be generalized to monotone and pseudomonotone operators
which are not potential operators.
(vi) In Section 25.9 we shall consider applications to nonlinear conservation
laws which allow man}' applications in various fields of physics.
(vii) Parallel to the minimum problem (53) on the entire space X we also
consider minimum problems of the form
f(u) = min!,
UE C,
where C is a convex subset of X. Then the solutions of this minimum
problem are also solutions of the variational inequality
<f'(uv - u)  0
for aU v e C.
(viii) In Part III we shall study potential operators in greater detail. In
particular, we shall consider eigenvalue problems for potential operators
and a duality theory which leads, for example, to two-sided error esti-
mates for the Ritz method.
Recall that a set C in a linear space (e.g., a B-space) is called convex iff
U, v e C and t e [0, I] imply (I - t)u + tv e C,
i.e.. if the points u and v belong to C, then the segment joining them also
belongs to C (Fig. 25.3). A functional
f: C ..... R
on a convex set C is called cont'ex iff
f«1 - t)u + tv)  (1 - t)/(u) + r/(v)'
for all t e [0, I] and all u, v e C, i.e., the chords. always lie above the curve
corresponding to f (Figs. 25.4(a), (b».
The functional f is called strictly convex iff
f«l - t)u + tv) < (1 - t)f(u) + tj(v),
for all t e ]0, I [ and all II, v e C with u :1= v, i.e., the interior points of th chDrds
lie properly above the curve (Fig. 25.4(a». The function f in Figure 25.4(b) is
convex, but not strictly convex.
Moreover, f is called concave (resp. strictly concave) iff - f is convex (resp.
strictly convex). As we will see in Part III, convexity is one of the most
important notions in mathematics and physics. For example, in many cases
energy is convex and en'ropy is concave.

508
25. Lipschitz Continuous. Strongl)' Monotone Operators
Figure 25.3
Classical Prototype 15.8. Let C be a cont'ex set in A, i.e., C is an interval, and
let I: C  iii be a differentiable fil'Jction. Then the ,(ollo\\'ing facts are ,vel'-
k'Jown:
(i) f;s convex on C iff I' is monotone on C (Fig. 25.S).
(ii) f is strictly cont'ex on C iff I' is strictly monotone on C.
(iii) If f is strictl} convex, then f has at ,nost one nJillil1Jal point on C.
(iv) If I: R ....IR is conve.V: and I(u) -. +00 as 1111 -. co holds, then f has a
minimum on IIi (Fig. 25.5).
The proof can be found, for example, in Fichtenholz (1972, M), Section 143.
In the following we want to generalize those results to B-spaces. In this
connection, we will obtain very simple proofs by reducing the general case to
/.
/'
./
./
/'
./
/'
/'
,/
f
.
u v
(a) strict1y convcx
(b) convex
/'
,/
/'
/'
/'
/'
/'
./
.
.-.
(c) strictly conca\'c
(d) conca\'c:
Figure 25.4

25.5. Monotone Operators and the C.alculus or Variations
S09
f
(a)
(b)
Figure 25.5
the Classical Prototype 25.8. To this end, we introduce the function
(,O(t) = 1«1 - t)u + tv)
for fixed u and v, where f is a functional on a linear space. Then cp is a real
function. Since the convexity off depends only on the values off on segments,
we immediately obtain the following result.
Lemma 25.9. Let C be a conL'e. set in a linear space (e.g., a B-space). Theil tile
follo\\'ing ''''0 (ondi'ions are equivalellt.
(i) The fUllctional f: C ..... [Ii ;S cOllvex (resp. strictly convex).
(ii) The real jrlnct;on cp: [0. 1] -+ R is cont'ex for all  v E C (resp. tp ;s slriftl.v
cont'ex on ]0, 1 [ for all II, v E C with U :F v).
The following result shows that the theory of monotone operators is a
natural generalization of the calculus of variations for convex functionals.
Proposition 25.10. Let f: C c X -+ R be a G-differelltiable functional on ,lie
cOllvex set C in tile real B-space X. Then the follo\\'ing t\\'O cOllditions are
ei/u;(,alen, :
(i) f i. COIIL'e. (resp. stricti}' convex).
(ii) I': C -+ X. is monotone (resp. stricti)' monotone).
Con,'ention. Rccall the following from Section 4.2. First let C be an open subset
of the B-space X (e.g., C = X). Then the functional f: C c X -+ R is called
G-differentiable iff it is G-difTerentiable at each point II E C. That i for each
u e C, there exists a functional a e X. such that
, . feu + tll) - feu) _ ( h)
1m - a,
,.-0 I
for all heX.
We set !'(u) = Q.
If C is an arbitrar}' subset of the B-space X, then f: C  X .... R is called
a-differentiable iff f is defined on an open neighborhood of C and f is
G-differentiable at each point II e C.

510
25. Lipschitz Continuous. Strongly Monotone Operators
PROOF. We fix u, v E C and set
cp(I) = feu + I(V - U», 0 < I < 1. (54)
Differentiation yields lp'(t) = /'(r4 + I(V - u»(v - u). Since f'(,,') e X., we can
also write
cp'(t) = <I'(u + t(v - u», v - u).
(55)
(I) Letf: C  R be convex. Then tp: [0, 1]  R is convex and ql is monotone.
From <p'(I)  tp'(O) we obtain that
<f'(V) - f'(u v - u)  0
for all u, v e C,
i.e.. f' is monotone.
(II) Let f': C -+ X. be monotone. If s < I, then
q>'(t) - tp'(s) = <f'(u + t(v - u» - f'(U + s(v - u) v - u)  0,
since f' is monotone. Hence ql is monotone. Thus, cp is convex and hence
[is convex. 0
The following proposition describes the connection between the minimum
problem (53) and the operator equation (52) above. At the same time we will
show how variational inequalities arise from variational problems.
Proposition 25.1 I (Abstract Euler Equation). Let f: C S X  R be G-
differentiable on the subsel C of the B-space X. Suppose thaI u is a solutio" of
the nJ;lJi,num problem
feu) = min!, u E C.
Then:
(a) If C is open, then u is a solution of the operator equation
f'(u) = O.
(b) If C is COIJVeX, then u ;s a solution of the tVlriational ;nequalil}'
(f'(II), V - u) > 0
for all v E C.
PROOF. We set q>(t) = feu + t(v - u) for fixed u, v E C.
(I) Let C be convex. If f: C  R has a minimum at II, then tp: [0, 1] -t R has
a minimum at t = 0, i.e.,
cp'(O) > O.
This implies
<f'(u), v - u) > 0
for all v E C.
(II) If C is open, then this inequality holds for all v in a neighborhood of u,
i.e.,I'(u) = O. 0

25.5. Monotone Operators and the Calculus or Variations
511
25.5a. A General Minimum Principle
Our next goal is to obtain existence theorems for minimum problems on
a-spaces.
Classical Prototype 25.12 (Theorem of Weierstrass). Let -00 < a < b < 00.
If f: [a,b].-. R is continuous, then f has a minimum and a maxinlunl on [a,b]
(Fig. 25.6(a».
Let f: M c X .-. R be a continuous function on a nonempty bounded
closed set M of a B-space X with dim X < 00. Then f has a minimum and a
maximum on M.
This is a classical generalization of Example 25.12. Note that X can be
identified with R. v for some N. Unfortunately, this result does not remain true
in the case where dim X = 00. Many of the troubles in the history of the
calculus of variations came from this fact. The deeper reason for this negative
result is based on the theorem that the closed unit ball in X is compact iff
dim X < 00. Thus, we need a notion which is weaker than continuity. This
notion is the weak sequential lower semicontinuity offunctionals. The follow-
ing definition is the most important definition in the calculus of variations.
Definition 25.13. Let f: M  X -+ R be a functional on the subset M of the
B-space X. Then f is caUed \\'eakl)7 sequentially lo\\'er semicontinuous on Miff,
r
/./
.
a b
(a) (b)
r
(c)
Figure 25.6

512
25. Lipschitz Continuous, Strongl)' Monotone Operators
for each II E M and each sequence (u.) in M,
UII U as n  00 implies f(u)  lim I(u,,).
11-00
EXAMPLE 25.14. Let f: [a, b] ..... R be a function with -00 < a < b < 00.
(i) If I is continuous. then / is also weakly sequentially lower semicontinuous.
(ii) The function f in Figure 2S.6(b) is weakly sequentially lower semi-
continuous, but f in Figure 25.6(c) does not have this property.
PROOF. We prove (i). Notc that in a linite-dimensional B-space weak con-
vergence and strong convergence coincide. Thus, if II,,U as n..... 00, then
I'" --. II and hence f(u) = limIt-a:. f(u n ). 0
The following theorem is the nlost important theorem in the calculus of
variations. It is motivated by Figure 25.6(b).
Theorem 25.C (Main Theorem on Minimum Problems). Sllppose that the
functiolJaI f: JW e X... R lIa. tile follo\ving '\\'0 properties.
(i) J\{ is a nonempt}f bounded closed convex set in tile reflexive B-space X.
(ii) f;s \,'eakl.v sequentially lower semicontiPJuous on M.
Then f lias a minimum.
In Proposition 25.20 below we will show that large classes of functionals
possess the property (ii). For example, each continuous convex functional
f: J\1 ... R on a closed convex set M in a B-space is weakly sequentially lower
scmicontinuous. Thus, we can replace condition (ii) by the following condition:
f is continuous and convex on M.
Corollary 25.15 (Uniqueness). A stricti}' convex functional f: M -.IR on a
convex set M has at most one minimal point.
PROOF. We set a = inf.,.; M f(u). Then there is a sequence (u,,) in M such that
f(u n ) -.  as n ..... 00. Since M is bounded and X is reflexive, there is a sub-
sequence, again denoted b)' (un)' so that u. u as n ... 00. The set M is closed
and convex. Hence u € M. By (ii),
f(u)  lim f(u.) = a.
....a:.
Therefore, f(u) = eX.
o
PROOF OF COROI.LARY 25.15. Suppose that f(u) = f(v) =  and U :/: v. Then
.(2- 1 (11 + v» < 2- 1 (f(u) + f(v» = .
This is a contradiction to the construction of eX above.
o

25.S. Monotone Operators and the Calculus of Variations
513
25.5b. The Main Theorem on Weakly Coercive Functionals
If the functional f in Theorem 25.C attains its minimum at an inner point u
of JW, then f'(u) = 0 by Proposition 25.11. The following coerciveness pro-
perties of ! guarantee this.
Definition 25.16. Let f: JW c X -+ R be a functional on the subset JW of the
B-spacc X.
(i) f is called coercive iff I(u)/llull -+ +00 as ilutJ -+ 00 on M.
(ii) f is called weakly coercive iff feu) -. +00 as IIr41i -. cc on M.
EXAJ.IPLE 25.17. Let a: X x X -. R be a strongly positive bilinear functional
on the B-space X. Then a is coercive.
PROOF. For aU u e X and fixed c > O,a(u, II) > C lIull 2 . Hence a(u, u)/llull -. +
as 11 r411 .... 00. 0
In contrast to Theorem 25.C. the set M can be unbounded in the following
theorem.
Theorem 25.D (Main Theorem on Weakly Coercive Functionals). Suppose
that the futlctional f: M c X -. R has the following tllree properties:
(i) J\I is a nonenapty closed cOPlvex set in the reflexive B-space X (e.g., M = X).
(ii) / is ,,,eakl)7 sequentially lower semicontinuous on M.
(iii) f is ,,,eakl)7 coercive.
Then f has a minimunJ on M.
By Proposition 25.20 below, assumption (ii) is satisfied if, for example, f is
convex and continuous on M.
CoroUary 15.18. If, in addition, M = X and f is G-differentiable on X, tlle'l tile
operator equatioPl !'(u) = 0 has a solution on X.
PROOF. Let Uo be a fixed element in M. Since f(u) -+ +00 as Uulj -. 00 we find
a closed ball B = {f4 e X: lIuli  R} so that Uo e B n M and
feu)  I(u o ) outside B n M.
Hence it is sufficient to consider the minimum problem for f on B (\ M. Then
Theorem 25.C yields the assertion. 0
Corollary 25.18 follows from Proposition 25.11.

514
25. Upschitz Continuous" Strongly Monotone Operators
25.5c. Criteria for the Weak Sequential Lower
Semicontinuity of Functionals
The following three standard examples show that large classes of functionals
are weakly sequentially lower semicontinuous.
Proposition 25.19. Let I: M c X  R be a filIJCtional on the closed set J\{ of
the B-space X ,,'itlJ dim X < 00. Then f is ,,'eakly sequentially lower senJi-
conti,ulOIlS if one of the following t,,'o conditions is satisfied:
(i) f is continuous.
(ii) f is lower sel1J;cont;nuous.
PROOF. We set
M, = {u e M:f(u)  r}. (56)
The point is that it follo\\'s from (i) or (ii) that the set J, is closed for each r
(cf. Definition 9.11).
If / is not weakly sequentially lower semicontinuous on M, then there exists
a sequence (u II ) in J'd such that u,, U as n .... ex) and
f(ll) > lim f(u,,).
" - ct.:
Hence there is an r so that r < feu) and u" E M, for all n  no. Since dim X <
00, we obtain U II  U as IJ  00; therefore, u e Mr. This contradicts r < f(u).
o
Proposition 25.20. Let f: M c X  IR be a functional on the convex closed set
M of the B-space X. Then f is \veakly sequentially lo\ver semicontinuous if one
of the following three conditions is satisfied:
(i) f is contilJuous and contx.
(ii) f is lower semicontinuous and cont'ex.
(iii) 1 is G-differentiable on M and I' is monotone on M.
PROOF. Ad(i), (ii). The set M, in (56) is closed and convex for all r.lfthe assertion
is not true, then there is a sequence (u,,) in M with 14"  U as n .... 00 and
feu) > lim feu,,).
" ... ex-
Consequently, there is an r so that r < f(u) and u. E M, for all IJ > "0' Since
M, is convex and closed, u eM,. This is a contradiction.
Ad(iii). We set q)(t) = feu + leV - u». Then tp: [0, t] .... R is convex and cp'
is monotone. By the classical mean value theorem,
lp(l) - cp(O) = q)'(8) > '(O),
o < 8 < 1,
.
I.e.,
I(v) > f(u) + <f'(u), v - u)
for all u, v e M.
(57)

25.5. Monotone Operators and the Calculus or Variations
515
If U"  u, then <f'(u), UtI - u) -+ 0 as n -+ 00. Hence
lim f(u.)  f(u).
o
.<JO
In Chapter 27 we shall show that important classes of quasi-linear elliptic
differential operators correspond to pseudomonotone operators which
generalize monotone operators. In the following, we want to show that this
class of operators also plays an important role in the calculus of variations.
By definition, an operator
A: M  X ...... X.
on the subset J\1 of the real B-space X is called pseudomolJotone iff, for each
u E M and each sequence (u,,) in M,
u,,u as n -. 00
and
lim (Au", u" - u) :5; 0
"-'<7)
imply
(Au,u - \v)  Jim (Au",u" - \v)
for all '" E X.
n-'
This definition seems to be obscure. However, in Section 27.2 we shall show
that pscudomonotone operators are quite natural objects. The prototype of
a pscudomonotone operator A: X .... X. on a real reflexive B-space X is
A = B + C,
where B: X -+ X. is monotone and continuous and C: X ..... X. is strongly
continuous, i.e., U II  u implies Cu" -+ Cu as n -. 00. Thus, sufficiently regular
perturbations of continuous monotone operators are pseudomonotone. In
particular, if f: X .... R is a C1.functional on the real reOexive B-space X, then
I' is pseudomonotone if f is a sufficiently regular perturbation of a convex
C I.functional.
Proposition 25.21. Let f: M c X -. R be a CI-functional on the open convex
set JW of the real B-space X, and let f' be pseudomonotone alul bounded.
Thera, f is \veakly sequentially lower semicontinuous on M.
PROOF.
(I) Let v,, v on JW as n -t> 00. The pseudomonotonicity of f' implies
lim (f'(v,,), v" - v)  o.
" -. IX)
Otherwise there would exist a subsequence, again denoted by (v,, such
that
lim <I' (v,,), VII - v) < o.
" -- <0
Since I' is pseudomonotone, we obtain lim ,,-. <f'(v,,), V n - v) > O. This
is a contradiction.

516
25. Lipschitz Continuous. Strongly tonotone Operators
(II) We set tp(t) = !(u + t(v - u». From
cp(l) - cp(O) = JOI cp'(l)dr
we obtain the ke}: relation
f(v) = feu) + JOI (f'(u + leV - u». v - 14) dr
for all U t v e M.
(58)
(III) Let 1I,,r4 on M as II -. 00. We set
v,,(t) = u + l(U II - u) for t e [0, I].
Then V,.(t) u on M as n --. 00. Let t > O. By (I),
,-1 lim <f'(v,,(t»), VII(t) - u) = lim <f'(v,,(t», U" - u) > o. (59)
,,-.
.. -e X)
From (58) we obtain that
feu,,) - feu)  II [(f'(v,,(t». 14" - 14) J- dl.
where we set [a]_ = min(a,O). Since I' is continuous, the integrand is
continuous with respect to t. Because I' is bounded, the integrand is
uniformly bounded. Finally, by (59), the integrand goes to zero as n .... cc.
Hence
lim feu,,)  feu).
o
,,oo
Further sharp criteria for the weak sequential lower semicontinuity of
functionals can be found in Section 41.4.
2S.5d. The Main Theorem on Convex Minimum Problems
Theorem 2S.E. Let f: X .... IR be a COllvex, continuous, and weald}' coercive
functional 011 tlU! reflexit B-space X. Then, f has a minimum on X.
1-'he millinaal point is unique if f is strictI}' COlavex.
PROOF. By Proposition 25.20, the existence and uniqueness of a minimal point
follows from Theorem 25.D and Corollary 25.15, respectively. 0
25.6. The Main Theorem on Monotone
Potential Operators
Theorem 25.F. Let f: X -. R be a G-differentiable functional on the real reflex-
ive B-space X ,,'ilia the follo,,'ing t"'o properties:

25.6. The Main Theorem on tonotone Potential ()pcrators
517
(i) I' is InOlJotOlJe on X.
(ji) I is \.,'eakl.v coercive.
The" :
(a) The "Ii,li",unl problem
f(14) = min!,
and the operator equation
U E X,
(60)
f'(u) = 0,
UE X,
(60*)
are equivalent.
(b) BotlJ problems have a solution.
(c) Iff' is stricti}' monotone on X, then the solr'tions of (60) and (60*) are unique.
PROOf. Ad(a). If u is a solution of (60), then u is also a solution of (60*) by
Proposition 25.1 J.
Conversely, let U be a solution of (60.). From (57) it follows that
f(u) + <.('(u), v - u) < .rev)
for all t' E X,
i.e., " is a solution of (60).
Ad(b). By Proposition 25.20, f is weakly sequentially lower semicontinu-
OUSt Theorem 25.D yields the assertion.
Ad(c). If /'(14) = I' (v) = 0, then <f'(v) - f'(u), v - u) = o. The strict
monotonicity of f' yields II = V. 0
The following proposition is an interesting special case of Theorem 25.F.
The key condition is
c5 2 f(u; It) > c( 111111) n/lU for all u, heX. (61)
Proposition 25.22. Suppose that:
(i) "r/ae !uncliollal f: X -+ R ;s G-differe"tiable on the real reflexive B-space
X, and the secoIJd lariation 1J'(U; h) exists for all II, heX.
(ii) There;s Q .(u,action c: R+ -.IR+ ,,ilh c(t) -. +00 as t ..... +XJ so tllal (61)
holds.
Then:
(a) The minimum problem (60) alld the operator equation (60*) are equitVJlenl
and both problems have a solut;oll.
(b) For all 14, v E X,
<f'(v) - f'(u), v - u) > c(tlv - 14n)lIv - 1111.
(e) If e(l) > 0 for aliI> 0, then f' ;s stricti}' monotolle 011 X and the solutions
of (60) and (60*) are unique.

518
25. Lipschitz Continuous. Strongly Monotone Operators
Corollary 25.23. If, in addition, C ;s a nonempt)' closed cOlJvex subset of X, tllell
the "Ji,Ji,,",m problem
f(lI) = min!,
and t he varia'iollal inequalit)'
ue C,
(62)
<1'(11), V - II) > 0
for all I' E C and fi:<ed u E C
(62*)
are equivalent and both proble"as I,ave a solution.
If ee,) > 0 for all t > 0, tl,en the solutions 0.( (62) and (62-) are u'lique.
PROOF. We set tp(t) = f(u + leV - u» for aliI e [0, I] and fixed u, VEX. Then
q>'(t) = </'(u + t(v - U», I' - u),
</,"(1) = 2f(u + t(v - 14); v - u).
(I) For s < t, the mean value theorem yields
11"(1) - q>'(s) = </,"(8)(1 - s) > O.
o < 3 < I.
Therefore, ql is monotone and hence f(J is convex. Consequently, f is
convex and f' is monotonc.
(II) It follows from cp'(l) - q>'(0) = cpN(.9), O < 8 < I, that
<f'(v) - f'(u), v - u) > c(1I v - 1111) II v - ull.
(III) The Taylor formula yields cp(l) = </,(0) + cp'(O) + !f(J"(a9), i.c., for u = 0,
1(1,1) > 1(0) + </'(0), v) + !c(dvll) nvll.
Since 1</'(0). (')1  IIf'(O)" IIvll, we obtain that f(v) -. +oc as IIvll  I.
Theorem 25.F yields the assertion. 0
Corollary 25.23 follows from Proposition 25.11 and Theorem 25.D.
Remark 25.24. The proof shows that Corollary 25.23 also holds if we replace
(61) with the condition
"(,)  c(U v - ull) II v - ull
for all t E [0, 1] and allll, ve C.
25.7. The Main Theorem on Pseudomonotone
Potential Operators
Theorem 25.G. LeI f: X -. R be a CI-functiollal on the real reflexit"e B-space
X with the following '\\'0 properties:
(i) f' is pseudomonotone and bounded on X.
(ii) 1 is weak'}' eoerciL.

25.8. Application to the Main Theorem on Quadratic Variational I ncqual i tics 519
TIlelllhe I1li,lil1U'I1' problem
f(II) = min!,
u EX,
has a solution \\7hich is a/so a solution of tile operator equation
/'(Ii) = 0,
Ii e X.
PROOF. By Proposition 25.21, / is weakly sequentially lower semicontinuous.
Theorem 25.D yields the assertion. 0
25.8. Application to the Main Theorem on
Quadratic Variational Inequalities
We consider the quadrdtic variational inequality
a(u, IJ - u)  b(v - Ii)
for all (,\ e C and fixed u e C.
(63)
and we make the following assumptions:
(H I) C is a nonempty closed convex set in the real H-space X (e.g., C = X).
(H2) a: X x X -+ R is bilinear, bounded, and strongly positive, i.e., a(u, Ii) 
C lIu 11 2 for all 14 e X and fixed c > o.
(H3) h: X -. IR is linear and continuous.
In the special case C = X. problem (63) is equivalent to the equation
a(u, v) = b(1,1) for all VEX and fixed u e X. (64)
We first consider the easier case that a(., .) is symmetric. To this end. we study
the minimum problem
2- 1 a(u, II) - b(u) = min!.
u e C.
(65)
Proposition 25.25. Suppose that (H 1) IhrofiglJ (H3) hold and tl(.. .) is syml1Jetric.
TIlell the variatiollal inequalil y (63) and the n.inimum problena (65) are equilJalent
alul botl, problems IJat,'f! a uIJiqlle solution.
Tile functional in (65) is cont"e.'< and "teakl}' coercive on X.
PROOF. We set /(Ii) = 2- 1 a(u, II) - b(u) and
tp(t) = f(u + I(V - u»),
for all t E IR and fixed u. VEX. A simple computation yields
tp"(t) = a(u - v, u - v),
.
since
I(u + tll) = 2- 1 a(lI, u) + ta(lI, I,) + 2- 1 ,2 a(h, Il) - h(u) - th(h).

520
2S. Lipschitz Concinuous. Strongly Monotone Operators
Hence
<f'(,, v - u) = '(O) = a(u, L' - u) - b(v - u),
cS 2 f(u;v - u) = cp"(O) = a(u - t"u - v)  ellu - v1l 2 .
Corollary 25.23 yields the existence and uniqueness result.
Finally, it follows from qJ"(t) > 0 that cp is convex, i.e.,! is convex on X. Since
f( u)  2 -1 C II U 11 2 - II b 1111 1111 ,
f is weakly coercive on X.
o
This proposition shows that the main theorem on monotone potential
operators (Theorem 25.F) gelleralizes the main theorem on quadratic varia-
tional problems (Theorem 18.A).
We now consider the general case, i.e., a(., .) need not be symmetric. The
point is that in this case we canllot apply variational methods. However, we
can use the same trick as in the proof of Theorem 25.8, i.e., instead of the
original problem (63) we consider the following equivalent problem:
(lltL' - u) > (zit' - u) - t[a(z, l' - u) - b(v - u)] for all l,.' E C, (66a)
z = U, II E C, (66b)
where I > 0 is fixed. As in the proof of Theorem 25.8, we will apply the Banach
fixed-point theorem.
Theorem 25.H. If (H I) ,"roug" (H3) hold, then the l,ariat;onal ;Ilequaljl) (63)
lias a unique solutiol!.
PRoo...
(I) Uniqueness. Let II and u be solutions of (63). Then. for all ve C,
a( v - II)  b(t' - u
a( u, v - Ii) > bel' - ii).
Choosing v = II and v = u in the first and second equation, respectively,
we obtain
-a(u - ", U - u )  0,
i.e., u = u.
(II) Existence. By Proposition 25.25, for each z e C, the variational inequal-
ity (66a) has a solution U E C. We set
" = Sz.
\Ve shall show that the operator S: C -. C is k-contractive. Then S has
a fixed-point u by the Banach fixed-point theorem (Theorem I.A). From
u = Su we obtain that u is a solution of (66), i.e., u is a solution of (63).
(II-I) Since a(., .) is bounded, there exists a linear continuous operator A:

2S.9. Application to Nonlinear Stationary Conservation Laws
521
x  X with
a(u, v) = (Aul v) for all 14. VEX.
Let 14 = Sz and u = Sz. Then (66a) yields
(ulv - u) > (zlv - u) - 1[(Azlv - u) - b(v - u)],
( u lv - u)  ( z lv - u ) - t[(Azl[ - Ii) - b(t, - iij].
Choosing t' = Ii and l = u in the first and second equation, respectively,
we obtain
-liu - ull 2 > -«I - tA)(z - z)lu - iij.
Since l(xl}')1  IIxllll}711, it follows that
lIu - uU S U(I - t A)(z - z ) II. (67)
(11-2) We set '" = z - z . From
II (I - t A ) \v 11 2 = II '" [12 + t 211 A \v 11 2 - 21 ( A '" I w)
we obtain
11(1 - tA)\\'1I 2 S k 2 11 w1l 2 ,
where k 2 = 1 + ,211AII2 - 2tc. By (61), Mu - ull < kllz - z ll. i.e..
IISz - 5%11  k II: - z II for all z, Z E C.
From cllull 2  (Aulu) < ilAulllr4Ij, we obtain c S IIAII. Thus, if we
choose t such that 0 < t < 2c/1I A i1 2 . than k < I, and hence the operator
s: C -+ C is k-contractivc. 0
In Part V we shall show that the famous problem of the filtration of water
through a dam leads to the variational inequality (63). Moreover, in Parts III
through V we shall show that many problems in elasticity, plasticity, gas
dynamics, heat conduction. control theory, etc., can be treated by using
variational inequalities which arc generalizations of (63).
25.9. Application to Nonlinear Stationary
Conservation Laws
We want to study the fundamental nonlinear stationary conservation law
div j = f on G,
u=g on o. G,
jn = h on 02G
(68)
with the current density vector
j = -a(lgraduI 2 )gradr4.
(69)

522
25. Lipschitz Continuous.. Strongly Monotone Operators
11
/
I
a.G
Figure 25.7
We assume:
G is a bounded region in [RN, N  2, with cG E CO. I . Moreover,
0 1 G and 02 G are disjoint open subsets of l1G such that
oG = OIG U 02G .
Let n denote the outer unit normal vector on aG (Fig. 25.7).
Parallel to (68) we study the variational problem
I (p(lgrad ul) - fil)dx + f '",dO = min!,
G 2G
" = 9 on 0 1 G,
(70)
(71)
where
1 I S:
P(s) = 2 0 «(I) dl. (72)
In the special case  = 1, \ve have pes) = s2/2 and the original problem (68)
passes to the mixed boundary value problem for the Poisson equation
-Au=J onG,
u = g on o. G,
- ou =,. on a 2 G.
all
(73)
If O 2 G = 0 and 0 1 G = 0, then we obtain the first and second boundary value
problem, respectively. Of course, in case 02G = 0 the boundary integral in
(71) drops out.
Consequently, from the mathematical point of view, problem (68) is the
simplest lJolJlinear generalizalioll of the classical Dirichlet problem. In Part V
Yle shall sho\\' that (68) comprehends many completely different physical
problems in hydrodynamics and gas dynamics (subsonic and supersonic now),

25.9. Application to Nonlinear Slalionary (.'onservalion Laws
523
electrostatics magnetostatics, heat conduction, elasticity, and plasticity (e.g.,
the plastic torsion of rods), etc.
In order to have an intuitive picture at hand let N = 3 and regard u(x) as
the temperature of a body G at the point x. Thenj in (69) is the current density
vector of the stationary heat flow in G. The function f describes outer heat
sources. The boundary conditions prescribe the temperature I' on the bound-
ary part 0 1 G and the heat flow through the complementary boundary part
02 G. Note that jn is the normal component of j. A detailed discussion of the
precise physical meaning of (68) can be found in Sections 69.1 and 69.2.
Equation (69) represents a constitutive laM' which depends on the specific
properties of the material. In the case where 2 == constant, the positive number
 is called the heat conductivity, and (69) is called the Fourier law of heat
conductivity. The general case (69) corresponds to a nonlinear constitutive
law, where the heat conductivity depends on the gradient of temperature. The
negative sign in (69) reflects the fact that heat flows from points with higher
temperature to points with lower temperature. The larger the gradient of
temperature is the larger is the heat flow.
Our plan is the following:
(i) We prove existence and uniqueness results which only depend on the
qualitative behavior of the material function a('). This allows an abun-
dance of applications to physical problems. Furthermore, this way, we
obtain a unified approach to many special results in the mathematical
and physical literature.
(ii) We show that Theorem 25.8 on Lipschitz continuou strongly monotone
operators can be applied to (68). Hence, for example, we obtain effective
projection-iteration methods for solving (68).
(iii) We show that Theorem 25.C (the general minimum principle) can be
applied to (68).
(iv) For sol\'ing equation (68) by a rapidly convergent iteration method,
engineers invented the so-called Kacanov method. We prove the con-
vergence of this method.
(v) We develop a nice duality theory for (68) which yields, for example,
two-sided error estimates for the Ritz method. In this connection, the dual
problem arises in a quite natural way, namely, it corresponds to a dr,al
constitutive law.
(vi) We show that the c01Jvexit}' and monotonicity of the function fJ in (72)
leads to a convex variational problem (71) and the corresponding Euler
equation (68) is elliptic.
The latter result gives us considerable insight into the structure of (68). In
Part V we shall show that subsonic now corresponds to (68), where fJ is convex.
The passage from subsonic flow to supersonic flow causes serious mathe-
matical difficulties since fJ loses its convexity and equation (68) changes the
type from ellipticity to hyperbolicity. In naturc, there occur shock waves (e.g.,
sonic booms caused by supersonic aircraft). In Section 25.5 we have seen that

524
25. Lipschitz Continuous Strongl). Monotone Operators
convex minimum problems are relatively easy to handle. In contrast to this,
there arise great difficulties if the functional loses its convexity. Exactly this
situation occurs in the case of transonic flow. In Part V we shall prove
existence theorems for transonic now via variational methods by using the
recent method of compensated compactness which overcomes the lack of
convexity (resp. compactness). In this connection, an additional so-callcd
entropy condition plays a fundamental role which ensures that, roughly
speaking, the second law of thermodynamics is not violated. We postpone this
until Part V. since in that volume we shall be able to understand completely
the physical background.
The follo\ving proofs are based on the abstract results which we have proved
in the preceding sections. Only the duality results (v) form an exceptional casC.
In this connection, we will use the abstract duality theory for monotone
potential operators which will be developed in Part III. This should help the
reader to obtain a complete picture with respect to the fundamental equation
( 68).
The key to our approach is the following result. We consider the function
y: R.... -. ai with
}'(x) = P(II),
where fJ: IR .... R is given by
I J "1
fJ(s) = 2 0 CX(I) dt.
umrna 25.26. Let the function : R+ -+ IR. be continuous. Then
(i"(.)lh) = a(lxI 2 ) (xlh) .for all x, h e Rl\'.
(a) If {l is (stricc/}t) convex, chen so is }'.
(b) If If is strongl)' nJoIJotone, i.e., there is ad> 0 so that
/l'(t) - {J'(s) > d(t - s)
for all real t  s,
tllen it' is strongly monotone, i.e.,
(,,'(x) - ;"()')Ix - J') ;:: dlx - }t1 2 for all x, )' e (RN.
For e,:(ample.. this condition is satisfied if ;s C 1 and P"(s) ;:: d > 0 on R..
(c) Let a be C 1 . If II is COllvex on ]a, b[. then tile quadratic form
},"(x)h 2 = 2t.r'(lxI 2 ) <xl/l)2 + a(lxI 2 )lhI 2 (74)
is positive on R N for all x with a < Ixl < b.
(d) If P' is Lipschitz continuous, i.e., there is all L > 0 so that
I P'(c) - If(s) I s LI t - sl
for all t, s E IR,
thell i" is Lipschil z continllolls.. i.e..
I}"(x) - }"(Y)I S 3Llx - }'I
for all x, y e R"'.

25.9. Application to Nonlinear Stationar)' Conservation La"'s
525
We give the proof in Problem 25.2. Note the following. If the function
fJ: R. ..... R+ is convex and monotone increasing, then the function
x..... (J(lxl)
is convex on R.'J, N > 1. In fact, for all x, y e R.'J and t E [0, I], we get
{J ( { I X + (I - I)}' I) s P( I ,- I + (1 - t U }f I )
< tP(lxl) + (1 - r)fJ(I)'I).
Lemma 25.26 is closely connected with this simple observation.
25.9a. Convexity and Ellipticity
We use Cartesian coordinates x = (... . . ,",) and set D, = a/a. as well as
Du : (D 1 U,. . . , D",r.),
.'1
DuDv = L DiuDlv.
i=1
"
IDul 2 = L fD i ul 2 .
il
For brevity, we write Du instead of grad u. Then the original equation (68)
becomes
'"
- L D,(<«IDu,2)Du) = f on G,
;'1
u = g on o. G,
au
- (IDrI12)- = h on 02G.
on
The variational problem (71) becomes
f. (P(IDul) - fu)dx + f. IIu dO = min!,
G :G
U = 9 on VI G.
Using (74). equation (75) can be written in the form
-('}'''(Du)D 2 )u = f on G.
In fact, it follows from (74) that
(75)
(76)
(77)
.\1
,"(Du)D 2 = 2oc(IDuI 2 ) L D.uDJuDjDJ
I.}!!!'
N
+ (IDuI2) L Dl.
l=1
Equation (77) is called elliptic (resp. weakly elliptic) at the point x iff the

526
25. Lipschitz Continuous. Scrongl)1 Monotone Operators
corresponding quadratic form
II  y"(Du(x»h Z
is str;ctly pos;tit-e (resp. positive) on (R'v. Hence from Lemma 25.26 we imme-
diately obtain the following result.
Proposition 25.27. Let: R+ -.lR i be C 1 and let u be a C 2 -solution of equation
(75).
f (J is convex ;n a neighborhood of ti,e point IDu(x)l, then ec/ualion (75) is
",'eakl)t elliptic at the point x \\,;th respect to u.
25.9b. The Classical Variational Problem
Proposition 25.28. Lei G be a boutlded region in R N such that (70) holds.
SI,ppose thaI the functions p, f, g, and hare sldTu';enll)' s,nootl,. Then eaclt
sufficientl}' .,;nlooth solut;oll II of tile t'ariational problenl (76) is also a solution
of ti,e bO"'1dary value problem (75).
PROOF. \Ve write (76) in the form
F(II) = min!,
u = 9 on 0 1 G,
and we set tp(t) = F(u + tv), where v is a sufficiently smooth function on G with
v = 0 on 0 1 G.
If" is a solution of (76), then q>'(O) = 0, i.e.,
J. ((IDuI2)DI,Dv - fv)dx + J. hvdO = O.
G C2G
Note that tr(s) = (s2)s. Integration by parts yields
J. AtJdx + J. , BvdO = 0,
G cG
(78)
\\' he re
A = - L D,((IDuI2)D,u) - J:
I
all
B = II + (IDuI2)-;-.
(in
In particular, equation (78) holds for all v E Ct(G). Hence A = O. Variation of
IJ then also yields B = O. 0

25.100 Projection-Iteration Method for Conscnoation Laws
527
25.9c. The Classical Variational Inequality
Let C be a convex set of sufficiently smooth functions. Inste(id of (76) we
consider now the minimum problem
f. (P(IDul) - fi.)dx + f. hr. dO = min!,
G r)G
U = g on 0 1 G, u e C.
(79)
For example, we may set
C = {r. e CI(O): IDul  c},
where c is a constant.
Proposition 25.29. Let G be a bounded region in R' suclJ that (70) holds. SllppOse
rlult tile !unctioPls (1, J: g, and " are sufficienll}t smooth and C is a convex !iet of
slljJicientl)t snlooth functions.
lJ- r. is tI sufficientl)' smooth solution of rile variational problem (79), the" u ;s
a 50111lion of tile variational inequalit)'
f. (IDuI2)Dr.(Dv - Du) - I(v - u)d't + f. h(v - u)dO  0 (80)
G 2G
for all sufficientl}' smooth fUPlctions v OIl G \\l;th
v = g 01' 0 1 G (Inti v e C.
PROOF. We write (79) in the form
F(u) = min!,
u = 9 on 0 1 G, u e C,
and we set cp(t) = f"(r. + I(V - u», where v = 9 on 0 1 G and v e C. If u is a
solution of (79), then tp: [0. I]  R has a minimum at t = 0, i.e.,
tp' (0)  O.
This yields (80).
o
25.10. Projection-Iteration Method for
Conservation Laws
We set
N
Lu = - L D((IDI.12)D,u)
i-I

528
25. Lipschitz Continuous... Strongly Monotone Operators
and consider the boundary value problem
Lu = f on G.
II = 9 on 0 1 G,
2 au
-(X(lDul ) an = h on 02 G .
(81 )
Recall that
1 f s 2
fJ(s) = 2 J 0 !X(l) dr.
The special case  :: 1 corresponds to the mixed boundary value problem for
the Poisson equation.
We make the following assumptions:
(H 1) G is a bounded region in R1\. such that (70) holds and OJ G #- 0.
(H2) The function : R... -+ R... is continuous and P' is strongly monotone
and Lipschitz continuous, i.c., there are numbers d > 0 and L > 0 so
that
/f(t) - P'(s) > d(t - s)
IIf(r) - !l'(s) I sLit - sl
for all real t > s,
for all t, .  o.
(H3) We set
x = {K' e W 2 1 (G): w = 0 on 0 1 G},
and we equip X with the scalar product
(ult.) = fG DuDvdx.
By A2(53c X becomes an H-space wjth respect to (.1.). More precisely,
the two norms lIu1l 1 .2 and lIullx = (ulu)I/2 are equivalent on X.
(H4) We are given
Ie X*, 9 e W 2 1 (2 (0. G), h e W21/2(02G)*.
These conditions are fulfilled if, for example, we have
Ie L 2 (G), 9 E W 2 1 (G), h e L2(OZG). (82)
(H5) Let (Y",) be a Galerkin scheme in X with
YIII = span { 'VI III' . . . , w..- m }.
Assumption (H4) will be discussed in Remark 25.32 below. We shall show
that, in some sense, condition (H4) is the most general condition which
guarantees that the right-hand side b of the generalized problem (84) below
belongs to the dual space X.. and hence (84) has a solution.

25.10. Projection-Iteration Method for COrLrvation 1.3\\'5
529
t
Q
/


Figure 25.8
EXAMPI.E 25.30. Condition (H2) is satisfied if the function a: IR. -. R. is C 1
and there arc numbers d > 0 and L > 0 so that
o < d  "(s) < L for all s > o. (83)
Since {f'(s) = 2a'(s2)s2 + (S2), condition (H2) is satisfied if, for example, the
function e«') has the qualitative behavior of Figure 25.8, i.e., a is monotone
and bounded, and
2(0) > 0,
lim a.' (52 )5 2 < cc.
.J- .. «.
If we regard (lgraduI2) as heat conductivity and 14 as temperature, then
from the physical point of view, such a behavior of a is reasonable.
Definition 25.31. The generalized problem for (81) reads as follows: We seek a
function u e W 2 1 (G) such that
a(lI v) = b(v)
for all veX,
14 = g on 0 1 G,
(84)
where we set
a(u.v) = fG a(IDuI 2 )DuDvdx,
b(v) = J. fvdx - J. hvdO.
G o:G
The precise meaning of the boundary condition "u = g on 0) G.. will be
discussed in Remark 25.32 below.
If all the functions are sufficiently smooth, then we obtain (84) by multiply-
ing (81) with veX and subsequent integration by parts.
Let ii e W 2 1 (G) be a fixed extension of the given function g: 0 1 G  IR in the
sense of Remark 25.32 below, i.e., iJ = 9 on 0 1 G.
The projectiolJ-iteral;on metl10d for the generalized problem (84) reads as
follows. F.or m = 1, 2, . . ., we seck a function
".,. E Y m + 9

530
25. Lipschitz Continuous. Strongly Monotone Operators
such that 110 = ii on G and
(rl",Iw...) = (u m - 1 1"',,") - t(l(I1"'-J' Wk...) - b('''t",)], k = 1, ..., mi. (85)
Since u'" = 9 + Lt Ct... Wi",' this is a linear system Cor the unknown real num-
bers c 111I' . . ., C m ',". If all the functions are sufficiently smooth, then integration
by parts shows that (85) is exactly the Galerkin method for the following linear
differential equation \vith respect to II",:
-Au". = -Au III _ 1 - t(Lu"'- 1 - f)
u.. = 9 on 0 1 G,
OU. _ 011"'-1 ( (I D 1 2 ) 011"'-1 I  G
 - iJ - t 2 "..._1 a + I) on 02 ·
Lon IJ II
on G,
(85. )
Theorem 25.1 (The Mixed Boundary Value Problem Cor Conservation Laws).
Assume (H 1) through (H5). Then:
(i) Existence and uniqueness. The generalized problel11 (84) lias a ullique
soilltion u.
(ii) Projection-iteration method. If t is a fi:(ed IIun,ber "pith 0 < t < 2d/9L 2 ,
then the sequence (II",), cOlastructed b}' the projection-iteration method (85)
abolJe. cont'erge.'\ to u in Wl(G) as m  00.
(iii) Linear problem. In tile special case  = 1, we obtain the ,ni.'{ed boundar}'
f.,'Qllle problem Jor tile Poisson eqllation. llere the unique solution u of (84)
salisfies the estinaale
II u II R'  co ns t ( II f II x. + II g II r + U I, II z. )
for all give'J f e X*, 9 e Y, h E Z*, \vhere
W = W 2 J (G), X = {\\: e W: ,,,. = 0 on 0 1 G},
Y = Wl,2(OI G Z = W 2 1J1 (02 G).
I" particular, for all give" f e L 2 (G), g e Wi,2(OI G). I, e L2(02G), \ve
have .r eX., 9 e Y, h e Z., llnd tile unique solution u of (84) satisfies tile
estimate:
"lIl1w < const(lI.fIl L :I(G) + I1gnWr:t(i\G) + IihIlLlC,12Gt).
Remark 25.32 (Interpretation of the Generalized Problem (84).
(a) We discuss the assumption 9 E Wi/ 2 (i\ G). By A 2 (49) and A 2 (51), each
function 9 e W 2 1 (G) has generalized boundary values g e W 2 1 .'2(oG), and \ve
have
flgll w I'2((iG)  constllgllw(G) for all g E W 2 I (G).
Conversely, each function 9 e W 2 Ij2 (oG) can be extended to a function

25.10. Projection-Iteration Method (or Conservation La\\'s
531
9 e W 2 1 (G) such that
9 = 9 on oG,
and II g lI..rltG) s constllgll W},l(cG) for all 9 e W 2 1 !l(OG).
By definition, a function 9: 0 1 G .... R belongs to the space Wi l1 (OI G) iff
it can be extended to a function 9 e W 2 1t2 (oG). We set
119 II Wl'2 «('1 G) = if 119 II W'l(CG)'
,
where the infimum is taken over all possible extensions of g. Recall that
0 1 G c: aG.
This \vay, W 2 1/2(C 1 G) becomes a real normed space if we identify two
functions whose values differ on a subset of a l G of surface measure zero.
The space Wl'2(C2G) is defined analogously.
(b) We show that the embcddings
W2J,'"2(oJG)  L 2 (c j G), j = 1, 2,
are continuous. For example, let j = 1. By A 2 (SI).
(J'G g2 dO )'i2  constllgll wr:(CG) Cor all 9 e Wl I2 (oG).
Hence
(L. G g2 dO ) 1(2  const IIgHwO:(('1 G) Cor all 9 e Wl 1 '2(oa G).
(c) We discuss the assumption f e X*. We first consider the special case
f e L 2 (G). The Holder inequality yields
fG fvdx  II fIl2I1vll.. 2 < coostll f1l21! vllx.
for all veX, by (H3). Thus,! generates the linear continuous functional
V 1-+ fG fvdx
on the B-space X. In this sense we write f e X*. Note that
II f II X. S const II f 112.
In the general case, f e X., the integral fGfvdx does not always make
sense. Ho\\'ever, we agree that IGfvdx denotes the value of the functional
f at the point v, i.e., we write
(J. v)x = fG fvdx
for all f E X*.
(d) We discuss the assumption h e Z*, where Z = W2112(C2G). We first con-

532
25. Lipschitz Continuous. Strongly tonoton Operators
sider the special case II e L2(02G). Since the embeddings
X  W 2 1 (G)  W 2 1 / 2 (oG) £ Z c L 2 (02 G )
are continuous, we obtain that
1 IIvdO  (1 112 dO ) 1.12 (1 V2 dO ) ll2
1G !G lG
< const ilia II L:(i':G) II f,'!1 z for all v E Z.
Thus, h generates the linear continuous functional
v...... 1 lav dO
a:G
on Z. In this sense we write II e Z*. Note that
IIlJllz.  const Ilhll'_lc.:G) for all h e Z.
In the general casc, II e Z., y,'e agree that J:G hv dO denotes the value
of the functional h at the point v. i.e., we \\'rite
(II, v>z = 1 IlvdO for all v e z.
ClG
Since the embedding X  Z is continuous by (a), it follows from II e Z*
that, for all t' EX,
I (h, t' >z I < II h II z.11 V III  const II h II z.1I t' II x ·
This implies heX. and
IIh 111'. S const IIhll z . for all II e Z*.
(e) We show that be X*. In fact, let Ie X. and " e Z*. By Definition 25.31,
b(v) = 1 fvdx - 1 hvdO.
G (; : G
Hence, for all t eX,
Ib(t')1 :: i<.t: V>x - (". v>zl  const( IIflix. + IIhllz.)lIvllx-
Thus, \ve obtain b E X. and
IIbli x .  const(llflix. + IIhll z .).
(86)
PROOF OJ: TIIORt 25.1 IN TilE LINF.AR CASF:. We shall use the main theorem
on quadratic minimum problcms (Theorem 22.A). Let a = I.
(I) Equivalent norm on X. Recall that X  W, where
W = W 2 1 (G) and X = {,,' e W: '" = 0 on 0 1 G}.

2S.10. Projection-Iteration Method for Conservation Laws
533
We equip X with the scalar product
(ulv) = fG DuDvdx for all u. VEX.
and we set
UuU x = (UIU)112 = (fG IDul 2 dx Yl2 .
lIullw = (fG lul 2 + IDU I2 dXYI2.
From A 2 (S3c) we obtain the important fact that the two norms 11.11 wand
1I'lix are equivalent on the subspace X of  Thus, X becomes an H-space
\vith respect to the scalar product ( '1. ).
(II) The equivalent problem. The generalized problem (84) reads as follo\vs:
a(u, v) = b(v) for all v E X,
(P)
u = 9 on 0 1 G,
UE
where 9 e Y = wi 12 (0 1 G) is given. According to Remark 25.32(a), we
choose a fixed function 9 e W such that
9 = g on 0 1 G and '1gl1 w < const IIgll y.
Then, problem (P) is equivalent to:
a(lI,v) = b(v) for all v E X, u e 9 + X.
Finally, letting \\' = U - g , we obtain that the original generalized prob-
lem (P) is equivalent to:
a(,,', v) = b l (v)
for all v E X and fixed '" eX,
(87)
where
b.(v) = b(v) - a( g ,v) for all VEX.
(III) We show that b 1 e X*. By (86), be X*. For all veX,
la( g . v)1 = fG D g Dvdx  II g llwllvllx'
Therefore, we obtain from (86) that
IIblll x.  const(lIfll x. + IIhll z . + 11911 w).
(IV) Existence and uniqueness. Note that a(\v, v) = (\vlv) for all \\', veX in the
case where 2 = 1. Thus, it follows from Theorem 22.A that equation (87)
has a unique solution '" and
II "'II x  const IIblli x. for all b l eX..
Consequently, the original problem (P) has a unique solution u.

534
2S. Lipschitz Continuous. Strongly Monotone Operators
(V) Estimates. By (I),
11'\-'11 w S const IIb l llx.
Noting that II = W + g, we obtain
ftul1... S const(lIb 1 I1 x . + IIUII...).
Since IIgll w < const IIgII" this implies
JluJl w :s; const(lIfx. + lj'JM z . + UgH,).
for all b a e X*.
o
PROOF OF THEOREttf 25.1 IN THE GENERAL CASE.. We shall use Theorem 25.8.
(I) The equivalent problem. According to the preceding proof, the general-
ized problem (84) is equivalent to
a( ,,', v) = b(v) for aU v E X and fixed '\-' E X, (87.)
where
a(w,v) = fG a(IDwI 2 )DwDvdx,
and b E X.. 9 e W as well as
v = v + 9 and W = M' + (j.
(II) The equivalent operator equation. By (81), (res) = a(s2)s and hence
tr(O) = o. Thus it follows from assumption (H2) that Ja(s2)1 < L for all
S E R and hence
I a ( w, v) I  L II '" II x II v 11 x
for aU w, v EX.
From (22.la), we obtain that there exists an operator A: X .... X* such
that
(A,,\v) = a( w,v)
for all 'v, VEX.
Consequently, problem (87*) is equivalent to the operator equation
A",. = b, we X. (87.*)
(III) Properties of A. According to Lemma 25.26 we obtain that, for all v, 'v,
Z EX,
a (w, v) = fG (y'(Dw)IDv) dx
as weJl as
a(v,v - w) - a(w, v - w) = fG ()"(DV) - y'(Dw)ID v - Dw)dx
> d fG IDv - D w l 2 dx = dllv - will

25.1 t. The Main Theorem on Nonlinear Stationary Conservation La\\-s
535
and
l a( v, z) - a(w, z)1 = fG ()"(DV) - y'(DW)IDz> dx
S 3L fG IDv - D w llDzldx S 3Lllv - wllxllznx.
This implies that, for all v, w eX,
(Av - A""v - \\')  dllv - wlli,
IIAv - Awlx. S 3Lv - \vllx.
Thus, A: X -+ X. is strongly monotone and Lipschitz continuous.
(IV) By Theorem 25.8, the operator equation (87..) has a unique solution
and the corresponding projection-iteration method (85) converges. In
connection with (85), note that u = \v + g, '" e X. 0
25.11. The Main Theorem on Nonlinear
Stationary Conservation Laws
In Sections 2S.9b and 2S.9c we have studied classical variational problems
and variational inequalities with respect to conservation laws. We now want
to prove the existence of generalized solutions for those problems. To this end,
we set
F(u) = J. (fJ(IDul) - fu)dx + J. hlldO
G aJG
and consider the variational problem
F(u) = min!, II e W Z 1 (G),
u = 9 on 0 1 G.
(88)
More generally, we consider
F(u) = min!, u e C,
u = 9 on 0 1 G,
where C is a convex subset of W Z 1 (G).
Problem (88) corresponds to the variational problem in Section 25.9b. If C
is a proper subset of W 2 1 (G), then (89) corresponds to the variational inequality
in Section 25.9c. Recall that
(89)
I J. S
P(s) = 2 0 (l) dr.
We make the following assumptions:

536
25. Lipschitz Contjnuou Strongly Monotone Operators
(H 1) G is a bounded region in [RN such that (70) holds and o. G :;: 0. We set
W = W 2 1 (G) and equip W with the equivalent scalar product
(ulv) = f. DuDvdx + f. uvdO
G IG
(d. A 2 (S3c», and we set lIuli = (ulu)I/2.
(H2) We are given
f e W*, 9 e W21/2(CI G), h E W Z 1 /2(C2 G)*. (90)
For example, this assumption is fulfilled if
Ie L 2 (G), 9 e W 2 1 (G), II E L 2 (02 G ).
In the general case (90h the integrals JG fu dx and JC:l G lIu dO are to be
understood in the sense of Remark 25.32.
(H3) We set
JW = {u e W: u = 9 on 0 1 G}.
Since the embedding W c: Wl' 2 (C 1 G) is continuous, the set M is closed
and convex in W. Let C be a closed convex set in W with M r\ C  0
(e.g., C = W).
(H4) The function : R+ -. R+ is continuous and fJ: R ... R is convex. There
is a number c > 0 so that (s) S 2c on R+, i.e.,
o S P(s) s cs z on R.
If C is unbounded (e.g., C = W), then there is a number a > 0 so that
(s) > 2a on R..., i.e.,
as 2  pes) on IR.
Figure 25.9 shows the typical behavior of« and fl.
Theorem 25.J. Under the assunJptions (H 1) through (H4 the (,'Qr;alional prob-
lem (89) has a soilltion.
If fJ ;s strictly cont'ex on R, then tile solution is IlIJiqlle.
PROOF. We set
b(u) = f. fudx - f. hudO.
G 2G
By Rcmark 25.32, b E W*.
1
.
fJ
Q
Figure 25.9

25.12. Duality Theory for Conscf"ation Laws
537
(I) The functional I:: W -+ R is continuous. In fact, we have the growth
condition
o s {J(IDul) S clDul 2 .
Hence the integral F(u) exists for all u E  If
(91)
u,. -+ u in W
as n..... 00 t
then ID"nl-+ IDul in L 2 (G) as n -. 00, It follows from (91) and the con-
tinuity of the Nemyckii operator (Proposition 26.6) that
P(I Dr4,,1) -. {J(IDul) in L. (G)
as n -+ 00,
i.e., F(u.)  f"(14),
(II) F is convex on w: since x...... P(lxl) is convex on A It- by Lemma 25.26.
Moreover, if fJ is strictly convex on IR. then x...... P(lxl) is strictly convex
on R'v and hence F is strictly convex on w:
(III) If C is bounded, then the assertion follows from Theorem 25.C.
(IV) Let C be unbounded. Then fJ(s)  a.... 2 on IR. For u e M. this implies
f. P(IDul)dx > a f. IDul2 dx
G G
 allull 2 - a f. g2dO
r.,G
and
F(u)  a lIull 2 - IIbliliuli + const.
Hence F(u) -+ +00 as lIuli -+ 00 on M, i.e.. F is weakly coercive on M.
The assertion follows now from Theorem 25.D for C ("\ M. 0
25.12. Duality Theory for Conservation Laws
and Two-Sided a posteriori Error
Estimates for the Ritz Method
We consider the original problem
F(u) = min!,
u e W 2 1 (G),
(92)
u = 9 on 0 1 G,
together with the so-called dual problem
F.(p) = max!. P E Lz(Gr,
(92a *)
fG pDu dx = b(u)
for all u e Wl(G) with u = 0 on 0 1 G. (92b*)

538
25. Lipschitz Continuous. Strongly Monotone Operators
Here, we set P = (PI'...' Pt.')' pDrl = LI Pi DiU, and
b(u) = I fu dx - I hu dO.
G cG
F(u) c: fG P(IDul)dx - b(u
F.(p) = - fG (P.(lpl) - pDjj)dx - b(g
where 9 = 9 on 0 1 G and
I s 1 I S 2
p.(s) = 0 fJ'-I(t)dt, pes) = 2 0 «(t)dt.
Here. fJ'-1 denotes the inverse function to fJ'(s) = a(s2)s. The function p. is
called the conjugate function to fl. This notion coincides with the general
definition of conjugate functionals in Section 51.1.
Condition (92b.) is the generalized formulation of the classical equation
N
- L DiP. = f
;=1
on G,
-np = h on 02G,
where n denotes the outer unit normal vector of aG. In fact, choose u e CI(G)
with u = 0 on 0 1 G. Then multiplication of the first equation in (93) with u and
subsequent integration by parts yield (92b*).
Recall that the classical Euler equation to the original problem (92) reads
as follows:
(93)
"
- L D,(<«IDuI 2 )D,u) = / on G,
1=1
u = 9 on a l G,
(94)
OIl
-«(lDuI 2 ) on = h on 02 G .
We shall show that the unique solution u of the original problem (92) and the
unique solution p of the dual problem (92*) are related by the equation
p = «(IDuI 2 )Du.
Regarding (93) and (94), this is a very natural result.
The classical formulation of the original problem (92) reads as follows:
I (P(IDul) - fu)dx + I hudO = min!,
G C2G
U = g on 0 1 G.
(95)
(96)

25.12. Duality Theory for Conservation Laws
539
The classical formulation of the dual problem (92.) reads as follows:
- fG p.UDul)dx = max!,
N
- L D i (<«IDuI 2 )D 1 u) == f on G,
.1
(96*)
iJu
-oc(lDuI 2 ) an = h on a 2 G.
This is motivated by (93), (95) and by the relation
f. pDg dx - b(g) = f. (pD g - fg )dx + f. h g dO = 0
G G a 2 G
if we suppose that the given function g: 0 1 G -. (Ii can be extended to a suffi-
ciently smooth function g : G  R such that 9 = 0 on 02G.
The classical Cormulation (96) and (96.) shows clearly a remarkable duality
which was first discovered for the Laplace equation by Trefftz (1927). Namely.
the side condition of the original problem (96) is given by the boundary
condition, whereas the side condition of the dual problem (96.) is given
by the Euler equation and the natural boundary condition of the original
problem (96).
EXAMPLE 25.33. Let « = const > O. Then
(J(s) = a.s 2 /2,
p.(s) = s2/2a..
In this case the original problem (96) corresponds to the mixed boundary value
problem for the Poisson equation.
We make the following assumptions:
(HI) G is a bounded region in Rl\' with (70) and a) G =I: 0. We equip W%I(G)
with the equivalent scalar product
(ulv) = f. DuDvdx + r uvdO.
G JC1G
We are given
Ie W%l(G)*. 9 e W 2 112 (Ol G), he W2IJ2(02G)..
Let 9 e W 2 1 (G) be a fixed extension of 9 to G. For example, by Remark
25.32, we can choose
Ie Lz(G).
9 e W:l(G),
he L2(02G)
with jj = g.
(H2) The function «: IR+ ... R+ is continuous and p is strictly convex on R.

540
2S. Lipschitz Continuous. Strongly Monotone Operators
There arc numbers Q, c > 0 so that 2a < a(s) < 2c on IR., i.e.,
as 2  fJ(s)  cs 2 on R.
(H2.) The function ex: R+ -. 1Ji+ is C I and there is a number d > 0 so that
Jr(s)  d 00 IR.
Theorem 25.K (Main Theorem of the Duality Theory for Conservation Laws).
(a) If (H J  (H2) hold, then the origilJal problem (92) and the dual problenJ (92.)
have a IIlJiqlle solution ii and p, respectively, and
p = Cl(IDuI 2 )D II
ainJOSl ever}J,,'IJere on G.
(97)
Moreover, )"'e hat
F.(p) S F.( p ) = F(ii) < F(II), (98)
for (ll U E W 2 1 (G) ,,,'ith u = 9 011 0 1 G and all p e L 2 (G)''i '\lith (92b*).
(b) If, in additio'J, (H2*) holds, the'l there ex;.ts a nu,,,ber do > 0 so tllat
do fG lu - ul 2 dx <  fG IDu - D u l 2 dx
 F(u) - F.(p), (99)
J'or all II, p ,,'it II the same properties as in (98).
Remark 25.34 (Error Estimates). Relation (98) yields two-sided error estimates
for the minimal value l:(iij of the original problem. For example, we can
compute u and p by a Ritz method for the original problem (92) and the dual
problem (92*), rcspecti\'ely. The Ritz method for (92*) is called the Treffiz
method.
Furthermore, relation (99) yields error estimates for the solution ii of the
original problem. The existence of do > 0 in (99) results from the fact that ( .1. )
in (H 1) is an equivalent scalar product on W 2 1 (G) and we have u = u on o( G.
PROOF. Theorem 25.J yields existence and uniqueness of a solution for the
original problem (96).
For the dual problem, we need the duality theory for potential operators
in Chapter 51. In particular. we will use Theorem 51.B. To this end, we set
X = {,,,. e W 2 1 (G): 'v = 0 on 0 1 G},
Y = L 2 (G)I'l,
and we equip Y with the scalar product
(plq)y = fG pqdx.
For bre\'ity, we write pq instead of (plq) = rf=1 p;q;. We identify Y. with ¥.

25.12 Dualic)' Theory for Conservalion Laws
541
(I) Function P*. By Definition 51.1.
fJ*(s*) = sup (5. S - pes».
E R
( 1 (0)
By assumption (H2). the function P': iii  R is strictly monotone and
surjective. Hence If is bijective. By Proposition 51.5, fl*' = (J'-t, i.e.,
p*(s) = f fl'-I(t)dt.
It follo\ys from (100) that
fJ*(s*) = .* s - fJ(s), s* = (J'(s)
and hence
P*(s.) = a(s2)s2 - P(s).
(II) Function i t *. We set
s = !J'-l (s*).
( 1 00*)
y(p) = PClpl)
for all P E (RN.
By Definition 51.1,
)'*(p*) = sup (p* P - l'(P».
pR-
(101)
By Lemma 25.26, I is strictly convex. By (H2), i' is coercive and CI. Hence,
the function p....... y(p) --- p* p is strictly convex and Yleakly coercivc. Con-
sequently, for each p* ERN, the maximum problem (101) has a unique
solution p and p. - )t'(p) = 0, i.e.. p. = a(lpI2)p, and hence
Ip*1 = a(p2)lpl = P'(lpl).
By (101), }'. (p.) = p* P - J'(p). i.e.,
i'*(P*) = a(p2)p2 - P(lpl), Ipl = /l'-I(lp*I).
Thus we obtain from (100*) the ke}' formula
}'.(p.) = (J*(lp*l) Cor all p. e R It '.
(III) Function <5*. We set <5(p) = 1(P + c) for all P e RaY and fixed C E R"'. Then
c5.(p.) = sup (p. p - (p»
peR JII '
= sup (p*q - ,t(q» - p.c
4 c: Aft'
= y*(p*) - p*C.
(IV) .[he dual problem. Let
H(p) = fG y(p + D g )dx - b(g)
and let '" = II - g. Then the original problem (92) reads as follows:
(P) H(D,,') - b(w) = min!, '" e X.

542
25. Upschitz Continuou Strongly Monotone Operators
By Theorem 51.B the dual problem reads as follows:
- H.(p) = max!, p e Y,
(p.)
(p, DW)r = b(w) for all \V E X.
(V) We compute H*. Let c5(p) = ,(p + Dg). By Problem 51.7, the formation
of the conjugate functional and integration can be interchanged. Hence
H*(p) = (fG <5(P)dX)* + b(jj) = fG <5.(p)dx + beg)
= fG h'.(p) - pD g )dx + bun
= fG (P*(lpl) - pD g )dx + b(g
i.e., (p.) coincides with the dual problem (92-).
(VI) We compute H'. For all p, q E Y,
(H'(p),q)r = fG y'(p + Dii)qdx,
.
I.e.,
H'(p) = }"(p + D g ) = (Ip + DgI 2 )(p + D g ).
By Lemma 25.26.
(H'(p) - H'(q).p - q)r > d fG Ip - ql2dx.
Now, Theorem 51.B yields the assertions. 0
25.13. The Kacanov Method for Stationary
Conservation Laws
We consider again the conservation law
-div(tX(IDuI 2 )Du) = f on G,
u=g on 0 1 G. ( I 02)
ou
-(IDuI2)- = h on 02G.
011
Around 1950, engineers began to apply the following iteration method:
-div[cx(IDu i I 2 ) Du i+IJ = f on G,
Ut+l = 9 on 0, G,
_«(lDUtI2) OUHI = h on 8 2 G,
an
(103)

25.13. The Kaeanov Method for Stationary Conscr\'ation Laws
543
where k = 0, I, ..., and Uo is given with 1'0 = 9 on 0 1 G. The advantage of this
method is that the unknown function "1:+1 is determined by a linear equation
and, in practice, the method converges rapidly.
This method is called the secant modulus method or the Kacanov metlwd.
In order to obtain a simple physical interpretation of this method. we regard
II as temperature. Then
j = -a(IDuI 2 )Du
is the current density vector of the heat flow and  is the heat conductivity.
By (103), the unkno\\'n approximation Uk+l is determined by the heat conduc-
tivity 2(IDu.12) which corresponds to the known approximation u,.
Equation (102) corresponds to the variational problem
f. (P(IDul) - fu)dx + f. hudO = min!,
G G
U == 9 on a 1 G.
(104*)
and the iteration method (103) corresponds to the quadratic variational
problem for UI:+ 1 , where U1+1 = g on 0 1 G and
f. (2-1a(IDutI2)IDul:+112 - fU A + 1 )dx + f. hU"+l dO = min!. (105*)
G C)G
Our goal is to prove the convergence of this method.
We set Y = W 2 1 (G) and
C = {u € Y: u = g on 0 1 G}.
Then (104*) and (105*) correspond to
F(u) - b(ll) = min!,
and
u e C,
(104)
2- 1 B(u,,; U Ic + 1 , u.+ I ) - b(u lc + l ) = min!,
respectively. Here, we set
U"+I e C,
( 105)
f. S
pes) = r 1 0 (t)dt,
b(u) = f. fu dx - f. hu dO,
G c 1 G
F(u) = fG P(IDul)dx,
8(u;v, w) = fG Qf(lDuI 2 )DvDwdx.
We make the following assumptions:
(H I) G is a bounded region in R N such that (70) holds and 0 1 G #: 0. We are
.
given
f e W 2 1 (G)*,
g e W 2 1 / 2 (OI G),
h e W21/2(02G)*.

S44
25. Lipschitz Continuous. Strongly fonotone Operators
I
..
..
Figure 25.10
For example, we can choose
Ie L 2 (G),
9 € W 2 1 (G).
II e L 2 (02 G).
(H2) The function : R. -+ R+ is C I and there are positive numbers a, c. d so
that
a  rt(s)  c, a.' (s) :s; 0,
for all s > 0 (Fig. 25.10).
(J"(s) = '(S2)S2 + a(s2) > d,
Proposition 25.35. Assume (H I). (H2). Tllen tile original t'arialional problem
(104) has a unique soilltion u.
Let 140 e C be given. Then, for k = 0, 1, . . . . tlae quadratic variational problem
(105) has a unique soilltion "1+1 and the Kacanov method COllt'erges, i.e.,
"Ie -. U in W 2 1 (G) as k -t> 00.
PROOF. This proposition is a special case of Theorem 25.L in the next section.
Using our considerations about conservation laws in the previous sections,
it is easy to check the fulfillment of the assertions of Theorem 25.L. We VIrant
to discuss this.
Recall that Y = W 2 1 (G) and
C = {'v e Y: \v = g on 0 1 G}, X = {'v € Y: Mt = 0 on i\ G}.
As in Section 25.10 we set
lIuli x = (fG IDul 2 dx Y/2 and lIuli r = (fG lul 2 + IDui l dx yr2.
It is important for our proof that 11.11 x and II.U r are equivalent norms on X.
In the following note that v, wee implies v - we X.
(I) It follows as in Remark 25.32 that b e Y*.
(II) The functional F: Y --. R. Let t/1(s) = 2 -I J (X(t) dt. Then
1-"(11) = fG r/1(IDul l )dx for all u € Y.
Since a s (s)  c for all s  0, it follows from Problem 25.4 that F: Y 

25.14. The Abstract Kaeaoov Method for Variatiooallncqualitics
545
R is C I and
(F'(u), v) = fG 2""(lDuI2)DuDvd.
= fG (lD"12)DuDvdx = B(u; u. v) for all u, v E Y.
By Lemma 25,26(b for all v, wee and fixed Po > 0, we get
(F'(v) - F'(w),v - w)  fG dlDv - Dwl 2 dx > Pollv - wll,
since 1I'li x and 11.11 r are equivalent norms on X.
(III) The functional B: Y x y x Y -. R, From a S a(s) < c for all s > 0 it
follows that, for all v, \V E C and fixed p > 0,
B(u;v - w.v - w) > fG alDv - Dwl 2 dx > pUv - wll,
an for all u, v, '" E Y, we get
IB(u; v. w)1  fG clDvDwldx S cUvllrllwllr,
by the Holder inequality.
(IV) The key condition. From (X'(s) S; 0 for all s  0 it follows that
oc(t)(t - s) s; J: oc(t)dt s; oc(s)(t - s).
for all 0 < s S; t < 00. This implies
pe,) - pes) s 2- 1 (<«S2)t 2 - (S2)S2),
for all St I e R. Hence we obtain the ke}' relation
F(v) - F(II)  2- 1 (B(I': v, v) - B(u: u, u))
(V) Theorem 25.L yields the assertions.
for all u, veX.
o
25.14. The Abstract Kacanov Method for
Variational Inequalities
We make the following assumptions:
(HI) Let C be a nonempty closed convex set in the real H-space Y (e.g.,
C = Y). Let beY. and Uo e C be given.
(H2) The functional F: C -+ R is G-difTerentiable. There exists a functional
B: C x Y x Y -+ R such that, for all u, v, w e C, we have the rcprcscn-

546
2S. Lipschi(z Continuous. Strongl)' Monotone Opercltors
tation formula
(F'(u), v - ",) = B(II; U, V - ",)
and the key inequality
F(v) - F(u)  2- 1 (B(u;v,v) - B(u;u,u»). (106)
(H3) For each u E e, the map
(v, ",) t-+ B(u; v, ",)
is bilinear, bounded, and symmetric from Y x Y to R. There are num-
bers p > 0 and b > 0 such that
I B ( u: v, ",) I <  II villi '" II
for all U E C, v, \V E 
and
B(u; \". - V, "r - v) > p II'" - 11I2 for all u, v, W E C.
(H3.) The operator F J : C .... Y. is continuous and strongly monotone, Le.,
there is a number Po > 0 such that
(fe,(u) - r(v),u - v)  Poilu - vil 2 for all u, ve C.
Theorem 2S.L ASSII"le (HI), (H2). (H3). Then:
(a) For k = 0, I, . . . , tile quadratic variational problenl
(Q)
2- 1 B(U1: IIA+I' Ut+l) - b(Ui+l) = min!,
Uk+l e C,
lIas a 1I11;qlle solution U"+I, a"d
B(Ut;UA;+J'V - UA+l)  b(v - U"+I) for all v e C. (107)
(b) If, ill addition, (H3*) holds, then tile origillal variational problem
(V)
F(u) - b(u) = min!,
ue C,
lias a IIlJiqlle solution u. Moreover, II is ti,e unique solut;on of the variat;olJal
inequalit }
(F'(u), v - u)  b(v - u)
for all ve C and fixed u E C, (108)
and the Katanov method COIUJerges, that i.
"Ie -. u in Y
as k -. 00.
Corollaf)' 25.36. Assume (H 1), (H2), (H3). Suppose that ti,e set C ;s compact and
that the nJap
u  B(u: v, \v)
is equiconlinuous OIl c.. \vith respect to all v, '" e Y.
1.hen tI,e sequence (Ui) has at least one cluster point and eacll such cillster
point u is a solutio" of the variational inequalit}' (108).
In Part V we shall show that Corollary 25.36 can be used to compute

25.14. The Abstract Kano\' Method ror Varia1iona11ncqualitics
547
transonic flow. In this connection, the compactness of C results from a
so-called entropy condition and the method of compensated compactness.
PROOF OF THEOREM 25.L. Ad(a). For fixed u e C, we set
H(t,') = 2- 1 B(u; v, v) - b(v) for all v e Y.
By (H3).
(H'(v), \,,) = B(u; v, w) - b(\v)
for all v, \" E Y,
and hence, for all v, '" e C,
(H'(v) - H'(\v),V - ",) = B(u;v - \",V - w)  pllv - \"11 2 .
It follows from Problem 25.3 that the functional H: C -+ R is weakly coercive,
strictly convex, and continuous. Therefore, the minimum problem
H(,,') = min!, w e C.
has a unique solution "', and (H'(\v), v - w)  0 for all v E C.
Ad(b). We set G(u) = F(u) - b(u). By (H3*) and Problem 25.3 the func-
tional G: C -+ R is weakly coercive, strictly convex, and continuous. Therefore,
the minimum problem
G(u) = min!, u e C,
has a unique solution u, and (G'(u v - u)  0 for all v E C, i.e., (108) holds.
Since F' is strongly monotone on C, the solution u of the variational
inequality (108) is unique.
(I) We set
g(u) = 2- 1 (8(ut; u, u) - B(u,,; u" fl.» + F(u.) - b(u).
By (106), we obtain the key condition
G(U'+l) < g(UIc+I). (109)
From the quadratic minimum problem (Q) we obtain min..cg(u) =
g(Ut+I). Hence
g(Ut"1)  g(Uk).
( (10)
This yields
G(Uk+l) S g(Ut+l) :s; g(u,) = G(u,).
Thus, the sequence (G(u,) is monotone decreasing. Since G has a mini-
mum on C, this sequence is also bounded below and hence it is conver-
gent. This yields
lim G(I' t + 1 ) - G(u,) = o.
,iX)
(II) The quadratic variational inequality (107) implies
G(II,) - G(Uk'-l) > G(uJc) - g(14,+I)
= b(UIc+1 - u.J - 2- 1 B(ut; U"+1' U'+I) + 2- 1 B(u,; I'I:, u,)
> 2- 1 B(UA; u t + 1 - UA,llt+1 - UA)
 2- 1 pllulc+1 - utll 2 .

548
25. Lipschitz Continuou Strongly Monotone Operators
Hence
lIui+t - Utll ... 0 as k... 00. (111)
(III) By the original variational inequality (108),
Poilu" - ull 2 s (F'(ut) - F'(uu, - 14)
S (/:'(u t ), Ut - u) + b(1I - UIt).
= B(Ut; U", 14" - u) + b(u - UA).
From the quadratic variational inequality (107) we get
B(u t ; U", 14" - u) + b(u - u,,) < B(u,,; U re - lIre +1 , U. - U + UI:+l)
+ b(U"+1 - u,,).
This implies
poHr4, - U 11.2  11111:+1 - UA; II (<5 lIut - U + ut.ll1 + IIbl!).
Hence lIu" - flll -+ 0 as k -+ X). 0
PROOF OF COROLLARY 25.36. The sequence (u,) lies in the compact set C. Hence
there is a subsequence (u t ,) with
u,,- -+ u
as k' -+ 00,
and U e C. By (Ill).
fl". +1 -. II
as k' -. 00.
Letting k' -. 00, it follows from the quadratic variational inequality
B(ut; U"+l' v - 1',+1) > b(v - u.+ 1 ) for all t' E C
that
B(u;uv - II)  b(v - u) for all ve C.
This is the original \'ariational inequality (108).
In this connection, use the equicontinuity of II t-+ B(ll; v, ",). This implies
B(u; L', ",) - B( u ; V, w) = B(u; v, w) - B( u ; VI w)
+ B( u ; v - V, \\1) + B(ii; v, w - ", )... 0
as u -. u, V -+ V, and ", -+ w .
o
PROBLEMS
25.1. Theorem of Toeplitz for real sequences. Let T = (I,}) be an infinite matrix of real
numbers lij which has the following three properties:
(i) T is a triangular matrix, i.e., tl) == 0 for j > i.
(ii) Ii} -. 0 as i -. aJ for each j.
(Hi) sup; L"'llt;JI < 00.

Problems
549
Let (a,,) be a given sequence of real numbers. We set
b. = I". a. + t. 2 Q2 + ... + I""a..
Show: If a" ..... 0 as II ..... 00, then also b. -. 0 as n -. 00.
Hint: a. Fichtenholz (1972, M), Vol. 2, Section 391.
25.2. Proof of Lemma 25.26.
Solution: We will make essential use of the Schwarz inequality
-lxll):1  + (xl)') S Ixllyl,
for all ..'=, y E R"o. We set
tp(l) = (x + II,).
Then
tp'(O) = (y'(x)lh) = a(lxl 2 ) (xlh),
rpAl(O) = },O(x)h 2 .
Note that Jj'(s) Ie :I(s2)s and aCt)  0 for all t  O.
(I) Proof of (a). Let fJ be (strictly) convex. Then 11' is (strictly) monotone. The
Schwarz inequality yields
(,'(x) - }"()')Ix - y) == a(l..'=12) (xix - y) - (I)'12)()'lx - )')
 [a(l..,=1 2 )lxl - a(IYI2)IYI](lxl- I}'I)  0,
for all x, }' E A. Hence i" is (strictly) monotone; therefore, }' is (strictly)
convex. by Proposition 25.10.
(II) Proof of (b). Let
fj'(s) - If(t) > des - I)
for all s > 1 and fixed d > O.
Then the (unction s...... /f(s) - tis is monotone and hence
SH pes) - 2- 1 ds J
is convex. Application of (I) to the corresponding function
x.... )'(x) - 2 -1 dlxl 2
yields
«i'(x) - dx) - (}.'()') - dY)lx - }')  0
and hence
(i'(x) - j'()')lx - }')  dlx - }'12.
(III) Proof of (c). Let fJ be (strictly) convex on ]a. b[. Fix a point x with
a < Ixl < b. The same argument as in (I) shows that}' is (strictly) convex
in a neighborhood of x. Consequently, for h -# 0, the function qJ is (strictly)
convex in a neighborhood of t = 0, i.e., fP"(O)  O.
(IV) Proof of (d). The inequality
IP'(I) - '(s)1 < LI' - sl
for all I, S e R +
means
I oc(t 2 )t - «(S2 )sl s LI t - sl
for all I, S E R..

550
2S. Lipschicz Continuous, Strongly Monotone Operators
This implies
1(t2)1 s L
for all t.
( 112)
We use the decomposition
(/'(x) - y'(y)lz) = (lxI2)(xlz) - (X(I)'1 2 )()'lz)
= (lxI2)(.,= - ylz) + , (113)
\\' here
 I: [(X(lxI 2 ) - (I)'I)] < }'lz).
The Schwarz inequality yields
I b I s I  ( I x I Z) - «( I }t 1 2 ) II }' II z I
= I a ( I x 1 2 ) ( I y I - I x I) + «( I x 1 2 ) I x I - rt ( I}':Z I ) I }' III z I.
By (112),
I I < 2Lllxl - 1}'llizl < 2Llx - Ylizi.
By (113
1(7 '(x) - }" ( ,,) I z) I  LI x - )' II z I + I  I s 3 LI x - )' II % I.
Hence li"(x) - i"(y)1 S 3Llx - }'I for all x, }' e R'''.
25.3. Coerc;a.,'eness of junctionals. Let C be a convex subset of a real H-space X. Let
F: C -+ R be G-differentiable and suppose that F: C ... X. is continuous and
strongly monotonc, i.e.,
(F'(u) - F(v),u - v)  cQu - vll 2
for all u, ve C and fixed c > O. Set G(u) :: F(u) - b(u), where b e X*. By Pro-
position 25.10, G is strictly convex on C.
Show that G is weakly coercive and continuous.
Solution: Set tp(l) .. F(u + t(v - u) for fixed u, v E C. From
q>(1) - q>(0) = f: q>'(t)dr
we obtain that
G(v) - G(II) = II (F(II + I(V - II» - F(II).v - lI)dl
+ (F'(u), v - u) - b(v - u)
c
 21111 - vll 2 - II F'(u) 0 IIv - ull - IIb IIv - ull. (114)
Hence G(v) -+ +00 as IIvll -. a:) on C.
The continuity of G follows from (114) by using the continuity of F' on C and
l(a,b)1  lIalll1bll.
25.4. 1}'pical properties of var;al;onal integrals. Let G be a bounded region in R'''.
N  I, and let Du = (D l u,..., D.llu), where x = (, l'.'" 'tt) and D, II;; OIOi'

Problems
SSI
25.4a. Special case. Let
F(u) = L .,(lDuI 2 )dx. (II S)
and let X = W 2 1 (G). Use the continuity of the Nemyckii operator (Proposition
26.6) in order to show the following:
(i) If "': [R.  R is continuous and we have the growth condition
1"'(1)1 < const( 1 + I)
for al1 I  O.
then the functional F: X -. R is continuous.
(ii) If, in addition, "': (R. -t IR is C 1 and
1.;'(t)1  canst
then F: X ..... II is C. and
(F'(u),v) = L 2""(lDuI 2 ) DuDvdx
for all t  0,
for all u, VEX. (116)
Solution: Ad(i). If u. ..... u in X as n --+ 00. then
DiU" ... DiU in LJ(G)
as II..... 00
for all i.
Since t/lUDuI 2 ) < const( 1 + IDuI 2 ) it follows from Proposition 26.6 that
.;(IDu,,1 2 ) ... \lfUDuI 2 ) in '''1 (G) as n... OC',
and hence F(u,,) -t F(u) as n ...... oc.
Ad(ii For fixed u, veX, set
fP(t) - L (IDu + tDvl 2 )dx
for all I e [ - I, 1].
Formal differentiation yields
ep'(t) = t 2""(lDu + tDvI 2 )(Du + tDv) Dv dx
for all t e [ - 1, t].
To justify this formal argument by means of the majorant criterion A 2 (2Sb),
note that, for all t E [ -1, t],
11/1'(1 Du + tDvI 2 )(Du + tDv) Dvl S const(IDul + IDvl) IDvl.
where the majorant function (IDul + IDvl)lDt'1 belongs to L1(G), according to
the Holder inequality and u, veX.
To prove the continuity of F': X -+ X., let h,(u) = 2""(lDuI 2 )D,u. Since
1""(1)1 s canst for all t  O. it follows from Proposition 26.6 that
U" --+ u in X
as n-.oo
implies that
h;(u,,) ... hi(u) in L 2 (G)
as n-+oo
for all i,
and hence
.'i
I(F(u.) - F'(u),v)1   IIh;(u.) - h;(u)1I 2 11vllx,
'-I

552
25. Lipschitz Continuous. Strongly Monotone Operators
for all veX, according to the Holder inequality. Therefore,
N
IIF'(u.) - P(u) II X.  L IIhi(u,,) - hj(u)lI l  0
'-I
as n... ex;.
2S.4b. Generalization. We no\\' consider the following morc general variational integral
F(u) = L 1.(u.Du.x)dx.
Let X II; Vpa(G), 1 < p < XJ. Show the following.
(i) If L: R........ x G --+ Ii is continuous and, for all u E  D ERN, X E G, we have
the gro\\'lh condition
IL(u. D. x)1  const(1 + lul P + 101').
then the functional F: X -+ R is continuous.
(ii) If, in addition, the function L is C. and, for all U E , D e RN,.t e G, \\'e have,
for the partial derivatives of L. the growth conditions
1 L.,(u, D. x)l, ILD...(u. O. x)1 s const(l + luI P -' + 101'-.).
then F: X -+ (R is C. and. for all u. f' eX,
(F'(u). v) = L I..,(u. Du. x)v + i 14D,.(u. Du. x)Djv dx.
Hint: Use the same arguments as in Problem 25.4a. In particular let p-. +
q-I = 1 and use Proposition 26.6 with p{q = p - 1 (continuity of the Nemycldi
operator from Lp(G) to L.(G)). in connection with the Holder inequality.
;= I,...,N,
References to the Literature
Classical \\'ork on Lipschitz continuous monotone operators: Zarantonello (1960).
Monotone potential operators: Vainberg (1956, M), (1972, M) Langenbach (1959).
(1966). (1976 M Browder (1966). (1970a), Ekeland and Temam (1974. M Gajewski.
Groger, and Zacharias (1974. M), Kluge (1979, M).
Projection iteration methods: Kurpel (1976, M) (comprehensive representation).
Gajewski and Kluge (1970" S), GajewskL Groger and Zacharias (1974, M), Langenbach
(1976, M), Scheurle (1977) (bifurcation theory).
Iteration methods: Browder and Petryshyn (1967, S Krasnoselskii (1973, M), Kluge
(1979 M) (general Toeplitz technique) (cr. also the References to the Literature for
Chapter I).
Variational inequalities: Lions (1968, M), (1969, M). Kinderlehrer and Stampacchia
(1980, M), "riedman (198 M).
Conservation laws and rheology: Langenbach (1959), (196S (1976, M) Gajewski
(1970), ( 1 970a), (1972), Gaje\\'ski, Groger, and Zacharias (1974. M).
Wpl-estimates for mixed boundary value problems on nonsmooth domains: Groger
( 1989).
Kaanov's method: FuCik, Kratochvil, and Necas (1973). Netas and Hlavak
(1981, M) (applications in clasticity
Kaanov's method for variational inequalities and transonic now in gas dynamics:
Fcistauer, Mandel. and Nceas (198S Gittel (1987).
Monotone operators and parameter identification: Kluge (1985. M

CHAPTER 26
Monotone Operators and Quasi-Linear
Elliptic Differential Equations
Without involving oursel\'es in the controversy over the fatherhood of mono-
tone operators, we \\'ould merely like to point out that this concept. among
others, appeared in the papers of Golomb (1935). Kacuro\'skii (1960) (influenced
b)' the papers of Vainberg), and Zarantonello (1960).1
Ha.im Brezis (1973)
The fundamental step in the theory of monotone operators was made by George
J. Minty (1962). 2
Mark Aleksandrovic Krasnoselskii and Pjotr Pjotrovi Zabreiko (1975)
Even before the creation of a general theory of monotone operators. ViJik (1961)
used certain monotonicity conditions in order to give new existence proofs for
quasi-linear partial differential equations of strongly elliptic type. . . .
The modern theory of monotone operators was developed in fundamental
papers by Minty (1962), (1963), Browder (1963), and Lerayand Lions (1965).
and in the works of a whole series of other authors during the following years.
Morduchaj Moiseevit Vainberg (1972)
The recently de\'eloped theor)' of monotone nonlinear operators from a
Banach space to its dual space can be considered most naturally as an extension
to non variational problems of the basic ideas of the direct method of the calculus
of variations. First of all, in practice, its simplest and most basic application is
to a class of nonlinear elliptic boundary value problems which is an extension
of the class of Euler-Lagrange equations of multiple integral problems of
general order, paralleling the application of Hilbert space methods for general
linear elliptic problems as an extension of the variational method for self-adjoint
problems.
Felix E. Bro\\'dcr (1966)
The truly new ideas are extremely rare in mathematics.
F olclorc
J Golomb (1935) and Vainbcrg (1956) "'orked on Hammerstein integral equations; Kacuro\'skii
(J 9(0) in\'estigated derivativcs of convex functionaJs.. and Zarantonello (1960) proved existence
theorems for strongly monotone. Lipschitz continuous operators on H-spaces (see Chapter 2S
 George J. Minty of Indiana University (U.S.A.) died in 1986. at the age of 56.
553

554
26. Monotone Operators and Quasi-Linear Elliptic Differential Equations
In this chapter, we study the operator equation
(E)
Au = b,
UE X,
where A: X -. X. is a monotone operator on the real a-space X. In order to
treat more general quasi-linear elliptic partial differential equations than those
in Chapter 25, \ve weaken the assumptions on the operator A, in contrast to
Theorem 25.8. In particular, we free ourselves of the assumption that A is
Lipschitz continuous or that (E) is related to a variational problem, i.e., A is
a potential operator. The main result of this chapter is the following:
If the operator A: X -+- X. is monotone, coercive, and lJeln;cont;nuous on the
real reflexive B-space X. then A is surject;L'e.
This means that for each b e X.., equation (E) has a solution. If A is strictly
monotone, then this solution is unique. The proof of this important existence
result in Section 26.2 is based on the following two ideas:
(i) We use a Galerkin method and solve the Galerkin equations by means of
the following existence principle: If f: Rr.- -+ R N is continuous and
(J(x)lx)  ° on oB,
where B = {x E R It -: IIxll < R}, then the equation
f(x) = 0,
xe B,
has a solution.
(ii) The convergence of the Galerkin method is proved by using the funda-
mental monotonicity trick (25.4).
Let N = 1. Then the existence principle in (i) follows immediately from
f(R) > 0, I( - R) S 0 and the intermediate value theorem of Bolzano. In the
case where N  2 we proved this existence principle in Section 2.4 as an easy
consequence of the Brouwer fixed-point theorem.
26.1. Hemicontinuity and Demicontinuity
Definilion 26.1. Let A: X .... X* be an operator on the real B-space X.
(a) A is said to be denJ;continuous iff
Un -+ I'
as n-.oo
implies Au,,-:' Au as n -+ rL:).
(b) A is said to be hemicont;nuous iff the real function
t..-. (A(u + IV), ".)
is continuous on [0, I] for all u, v, we X.

26.1. Hemicontinuity and Demicontinuity
555
(c) A is said to be strongly continuous iff
u u
II
as ,,-. 00
implies Au" ... Au as n ... 00.
(d) A is said to be bounded iff A maps bounded sets into bounded sets.
These definitions \vill be used frequently. Demicontinuity, strong continu-
ity, and boundedness can be defined entirely analogously for operators A:
X -. Y, where X and Yare B-spaces. For a more convenient formulation of
the following results, we make the following general assumption:
(H) Let A: X -. Y be an operator where X and Yare real reflexive B-spaces.
We first of all investigate the relation between strong continuity and
compactness.
Proposition 26.2. Under the assumption (H), the lollo\,,;ng 1\\'0 assertions are
valid:
(a) A is strongly continuous implies A ;s compact.
(b) A is linear and compact implies A is strongl)' continuous.
In (b) \"e do not need that the 8-spaces are reflexive.
We treat the proof in Problem 26.1. We next consider demicontinuous
operators.
Proposition 26.3. Under ti,e assunlplion (H), tlU! lollo\"ing 1\"0 assertions are
valid :
(a) A is dem;continllous implies A is 10 call}' bounded.
(b) A is demicont;nuous iff A is continuous as an operator from X into Y, in the
case where X is equipped \\.;th the nornl topolog}' arId Y is equipped with the
weak topology.
The reader will find the proof in Problem 26.2. Finall)', we study the
important continuity properties of monotone operators.
Proposition 26.4. Let A: X -. X. be an operator on ti,e real B-space X. Then:
(a) If A is monotone, then A ;s locally bounded.
(b) If A is linear and nwootone, then A is continuous.
(c) If A is monotone and hemicontilluous on the real reflexit'e B-space X, tllen
A is demicontilluollS.
The local boundedness of monotone operators plays an important role in
the theory of monotone operators.

556
26. ionotonc Operators and Quasi-Linear ElliptIC Differential Equations
PROOF Ad(a). Local boundedncss of A means that for each u e X there exists
a neighborhood V(II) such that A(U(14» is bounded.
Let A be monotone. Assume, to the contrary, that A is not locally bounded.
Then there exist a point 14 E X and a sequence (II,,) with
14"  U
and
II Au,,11 -. 00
as n ..... oc,..
Without loss of generality, let r4 = o. We set
a.. = (1 + II Au" 111111" II) -I .
It follows from the monotonicity of the operator A that
:t a.. (Au lI , v)  all ( (Au", 14,,) - (A( + v), 14" + v»
< a"(IIAu"lIlIu,,h + Ii A( + v)lIlIu lI + vII).
Therefore,
sup J(anAu",v)1 < 00
II
for all v EX.
According to the BalJach-Steinl,aus tl,eorem Al (35a), there exists a number N
such that
sup lIa"Au,,1I  N.
"
We set b ll = iI Au"lI. Then
b.. S a;l N = (1 + b"lIullll)N
for all II.
Since 1114,,11 -+ 0 as n -+ 00, the sequence (b.) is bounded. This contradicts
UAu.1I -+ :X) as n -+ 00.
Ad(b). Consider statement (a) and note the fact that a linear locally
bounded operator A is bounded on a neighborhood of zero and thus also on
the closed unit ball. This means that II Aull  const 111411 for all II e X, i.e.. A is
continuous.
Ad(c). Let u..  U as n .-. 00. Since (u,,) is bounded, the sequence (Ar4,,) is also
bounded, by (a).
Let Au".  b as n  00 for a subsequence (u...) of (u..). Then
<Au.., 14", > -. (b,u) as n -+ cc.
The monotonicity trick (25.4) gives Au = b. Hence, the convergence principle
(Proposition 21.23(i» yields
Au"  Au
as n  00.
o
26.2. The Main Theorem on Monotone Operators
We consider the operator equation
Au = b,
u EX,
(I)

26.2. The Main Theorem on Monotone Operators
557
together with the corresponding Galerkin equations
a(u lI , \Vi) = (b, Wk), k = 1, . . ., II.
In this connection, let
(2)
a(ll, t') = <Au, v),
X" = span { \".. , . . . , w" } .
We seek u" EX", i.e..
"
U" = L C'n "'A'
A=1
\vhere the coefficients Cbt are unknown real numbers.
Theorem Z6.A (Browder (1963), Minty (1963». Let A: X -t X. be a II'OIJOIOne,
coercive, aIJd hemicontinllous operator O,J tile real, separable, reflexive B-space
X. Assume {\v., "'2'. . . } is a basis in X. Then the lollon,tiny assertions hold:
(a) Solution set. For each b E X.. equatioll (1) lias a solution. Tile solution set
of ( 1) is bounded, COllve.,'(, alul closed.
(b) Galerkin method. II dim X = , then for each II e N. the Galerki1l equa-
tion (2) has a solution u" E X" aIJd the sequellce (un) has a \veakl)7 convergent
subseque'lCe
u,,' -.... u in X
as II -. 00,
,,'/Jere u is a solution of tile original equation (1).
(c) Uniqueness. If tl,e operalor A is striCll), monotone. lhen eqllatiolJ (1) (resp.
equatioll (2) is ulliquely solvable in X (resp. X,,).
(d) Inverse operator. If A ;s strictly monotone. then the in.,erse operator A-I:
X. -t X exists. Tllis operalor is strictly monotone, deIJJicontinuous, and
bounded.
If A is uniformly monotone. thel. A -I is continuous.
If A is strongl}' IJIOllotone, thell A -I is Lipschitz cOllt;nuous.
(e) Strong convergence of the Galerkin method. Let dim X = 00.11 the opera-
tor A is striCll) Inolaotone. tllen the sequence of Galerkin soilltions (u ll )
cont'erges weakl}' in X to the unique solution u of eqllatiolJ (I).
If A ;s ulliform/j1 nwnOlone, then (u..) converges slrolagly in X to the unique
Solul;on u of (I).
(f) Nonseparable spaces. If X ;s not separable, then the assertions (a), (c), and
(d) ren,a;n true.
PROOF. The basic idea of our proof is the following:
(i) We solve the Galerkin equations (2) by means of Proposition 2.8 which
follows from the Brouwer fixed-point theorem.
(ii) The convergence of the Galerkin method is based on the monotonicity
trick (25.4).
The monotonicit}' of the operator A is essentially used in (ii). We need the
coerciveness of A to get a priori estimates for the Galerkin solutions.

558
26. Monotone Operators and Quasi-Linear Elliptic DifferentiaJ Equations
Proof of (b).
Step 1: Solution of the Galerkin equations.
We set
g(u) = (Au - b, II),
The operator A is coercive, i.e.,
9(1I)/llull -. +00
g.(u) = (Au - b. \Vt).
as II u II -. 00.
Consequently, there exists a number R > 0 such that
g(u) > 0
for all u: lIu II  R.
(3)
This is the key condition.
The Galerkin equations read as follows:
(G)
9,(11,,) = 0,
II" EX",
k = 1, 2, . . . , n,
that is
"
u" = L Cleft W,.
'=1
Thus, (G) is a nonlinear system of real equations with respect to the numbers
Cln, ..., c"". By Proposition 26.4(c), the operator A is demicontinuous. Hence
the functions
u..... gk(U)
are cODtinuous on X.
In particular, the functions 9, in (G) are continuous with respect to
(c. II .... ,ClUJ). From (3) it follows that, for all u,. e X. with 1111,,11 = R,
.
L gt(II,,)Ctn = g(u n ) > O.
k-l
By Proposition 2.8. the Galerkin equation (G) has a solution.
Step 2: A priori estimates.
If Un is a solution of the Galerkin equation (G then
g(u.) = O.
From (3) it follows that
lIu,,1I < R
for all n.
(3.)
If u is a solution of equation (I), then g(u) = 0, and (3) implies
111111  R.
Step 3: Boundedness of (A un).
By Proposition 26.4(a), the operator A is locally bounded, i.e., there exist
positive numbers r and  such that
IIvll < r implies flAvll  b.

26.2. The Main Theorem on Monotone Operators
SS9
The operator A is monotone; therefore,
(Au,. - Av,u. - v)  o.
By the Galerkin equations (2),
(Au,., u,.) = (b, u,,)
for all n.
Hence
I (Au.., u,.) I  II bll It u,,11 S lib II R
by (3.). The definition of the norm in X. yields
IIAu,,1I = sup ,-1 (Au", v)
11...11 =r
S sup r-I«Av,v) + (Au",u.) - (Avtu,,»
,,'II=r
for all n,
 r-'(c5r + UbliR + R).
Step 4: Convergence of the Galerkin method.
The B-space X is reflexive. Thus, the bounded sequence (u..) has a weakly
convergent subsequence, again denoted by (u,,), i.e.,
UIIU in X
as n -. 00.
From the Galcrkin equations (2) it follows that
lim (Au., w) = (b, w)

for all", e U X".
.=1
(4)
"QC
Since U" X" is dense in X and (Au..) is bounded in X., relation (4) holds for
all ,\1 EX, i.e.,
Au -2a.b in X.
.
as n... OOt
by Proposition 21.26(c), (f). Furthcrmorc t by the Galerkin equations, we
obtain
lim (Au",u,,) = lim (b,u,,) = (b,u).
(5)
,,ao
."'00
By the monotonicity trick (25.4), it follows from
u,. -2a. u in X as n.... cx::,
Au.b in X.
as n -. 00,
and from (5) that Au = b, i.e., u is a solution of the original operator equation
(1 ).
Proof of (a). Let S be the solution set of equation (I) for fixed b e X*. This
set is nonempty by Step 4 above.
(I) S is bounded by Step 2 above.
(II) S is convex. To prove this, let u., U2 e S, i.e.,
AUi = b, i = 1, 2.

560
26. Monotone Operators and Quasi.Linear Elliptic Differential Equations
For u = ' 1 U 1 + '2U2 and 0 < '1' '2 < 1, '. + t 2 = 1, there follows the
inequality
2
(b - Av,u - v) = L 'f(Au, - AV.Il. - v) > 0,
1=1
for all VEX. The monotonicity trick (25.4a) yields Au = b, i.e., U E S.
(III) S is closed. Indeed. from Av" = b for all nand V n --. U as n .... 00 it follows
that
(b - Av,u - v) = lim (Av n - Av,v" - v) > 0,
,,-
for all VEX. The monotonicity trick (25.4a) yields All = b.
Proof of (c). Let the operator A be strictly monotone. By the uniqueness
trick (25.5 the equation Au = h has at most one solution u.
The same argument yields the unique solvability of the Galerkin equations.
Pr()of of (d). Let the operator A: X ... X* be strictly monotone. Then A is
injective by statement (c). By (a), the operator A is surjective. Hence A is
bijective, i.e.. the inverse operator A-I: X. -. X exists.
(I) A -1 is strictly monotone. Indeed, we get
(b - b, A- 1 b - A- 1 b ) = (Au - A u, u - u ) > 0 for u  u.
(II) A-- 1 is bounded. This follows from the coerciveness of A as in Step 1
above.
(III) A-I is dcmicontinuous. For, let v" = A- 1 b" and let
b n -. b
as n --. 00.
From the boundedness of A -I there follows the boundedness of (v,,).
Moreover, from v.....,.,.. v as n -. 00 it follows that
(b - A\\o', v - w) = lim (b., - Aw, V"' - \\0')  0,
" -. 'XI
for all '" e X. The monotonicity trick (25.4a) yields Av = b, i.e., v = A -I b.
Hence the convergence principle (Proposition 21.23(i» ensures that
v -..v
"
as n -. 00.
(IV) Let A be uniformly monotone. By (25.15), A is stable i.e.,
IIAu - Avll  a(lIu - vII) for all  veX,
\\'here the function a: R+ --. R. is strictly monotone and a(O) = O. This
implies the continuity of A-I: X. -. X. If A is strongly monotone, then
a( III' - vII) = clill - vII, C > o. Therefore, A -1 is Lipschitz continuous,
.
I.e.,
IIA- 1 b - A- 1 dll  c- I Jib - dB for all b, d e X*.
Proo.( o.(e). This follows immediately from the convergence tricks in (25.14).
Proof of (f). Suppose that X is not separable. Then we prove the convcr-

26.3. The Nem)'ckii Operator
561
gence of the Galerkin method by means of M-S sequences. which we intro-
duced in the Appendix of Part I.
To be precise let  = {Y} be the system of all the finite-dimensional sub-
spaces Y of X. We define an order relation on  by means of
y  Z iff Y s; z.
Then  is a directed set in the sense of AI (16). I n fact, if Y. Z e 1:, then 1':
Z  span(Y u Z).
For each Y e _ we now consider the corresponding Galerkin equation and
as above we obtain a solution Ur instead of u". Then (Uy) is an M-S sequence
which is bounded in the reflexive B-space X. Since each closed ball in X is
weakly compact, there exists an M -S subsequence (ur') with
Uy'  u
according to A I (17f). We now proceed as in the proof of (b) above. D
26.3. The Nemyckii Operator
In order to be able to apply Theorem 26.A to differential and integral equa-
tions, we require the properties of the so-called Nemyck;; operator F defined by
(Fu)(x) = I(x, u. (x),..., u,,(x»
(6)
with U c (u 1'. . . , u II ). Thus. F results when one replaces all the variables II} by
Uj(x) in I(x, u 1'. . . , u,,). We formulate the following conditions:
(HI) Caratheodor}t condition. Let f: G x (Ji" -+ R be a given function, where
G is a nonempty measurable set in RJ\- and n, N > 1. Moreover. the
following hold:
x f(x, u) is measurable on G for all U e frI;
u..... !("y., u) is continuous on Aft for almost all x E G.
(H2) Gro\vtlJ condition. For all (x, u) e G x iii",
II
If(x, u)1  a(x) + b L I U;IPi/4.
;-1
Here, b is a fixed positive number, the function a E Lq(G) is nonnegative,
and 1  q, Pi < 00 for all i.
EXA),IPLE 26.5. Condition (H I) is satisfied in the case where I: G x (1(" -+ R is
continuous.
Proposition 26.6. Under the assumpt;o1Js (H 1) a,ul (H2), the Nemyck;; operator
"
f": n L,,(G)  L 4 (G)
lei

562
26. Monotone Operators and Quasi-Linear Elliptic Differential Equation..
is continuous and bounded with
"
}1FIIII. < const( lIalL, + L u;1I :"4)
1=1 '
(7)
..
for all II e n L,.(G).
.-1
Before proving this, we consider the special case '1 = 1, i.e., we consider a
function f: G x R --. R. Then the Nemyckii operator F is given by
(Fu)(x) == f(x, u(x».
In particular, we arc interested in the case I:: X -+ X., where X = L,(G). This
corresponds to (HI) and (H2) with n = I. p = PI and p-l + q-l = 1, i.e.,
p/q = p - 1. More precisely, \ve make the following assumptions:
(AI) Caratheodor}' cOlulit;olJ. Let f: G x R -+ R be a given function, where
G is a nonempty measurable set in R'v, N  I. Suppose that:
x t-+ f(x.. u) is measurable on G for all U E R;
u...... f(x, u) is continuous on R for almost all x e G.
(A2) Gro\\,th condition. Let 1 < p, q < 00 and p-I + q-I = t. Suppose that
there exist a nonnegative function a e L.(G) and a fixed positive num-
ber b such that
If(x, u)1 s a(x) + blul,-I for all (x, u) e G x R.
(A3) Monotonicit} of ,(. The function f is monotone with respect to u, i.e.,
f(x, II) S f(x, v)
holds for all II, v E R with u  v and for all x E G.
(A3*) Strict monotonicity of f. The function f is strictly monotone with respect
to u, i.e.,
f(x.lI) < f(x, v)
holds for all II, v e R with II < v and for all x e G.
(A4) Coerciveness of f. For a fixed number d > 0 and a fixed function
9 E L1(G),
f(x,u)1I > dllli P + g(x) for all (x. u) e G x R.
(AS) Positivit}' of f. For all (x, u) e G x Ii,
f(x.. u)u  o.
(A6) As)'mptOlic positivity of f. There exists a number R > 0 such that
f(x, u)u  0
holds for all (x, u) e G x R with 1111  R, and meas G < oc.
Let X = L,(G). Then X. = L 4 (G).

26.3. The Nem)'Ckii Operator
563
Proposition '1.6.7. Under tile assumptions (AI), (A2), the following are valid:
(a) Tile NenJyckii operator F: X .... X. is continuous and bounded ,,'ith
IIFullq s const( naUf + lI u ll;-I)
for all u e X
and
(Fu.u)x= fGf(X,U(X»U(X)dX for all ueX.
(b) (A3) inaplies F is monotone.
(c) (A3*) implies /: is strictly monotone.
(d) (A4) ilnplies F is coercive and
(Fu,r,>X > dllull" + fG g(x)dx for all UE x.
(e) (A3.) and (A4) impl)' F satisfies the condition (5)+, i.e., il follo\vs from U"  u
in X as II -. 00 and
lim (Fu,. - FU t u. - u)  0
"  CIC
that II" -. u in X as n -. a;).
(f) (AS) implies
(Fu t u>x  0
for all U EX.
(g) (A6) implies
(Fu't u)x  -c
where c is a positive constant.
for all u e X,
In Propositions 32.44 and 41.10 we will prove the following important
additional results. IrCAI), (A2), and (A3) are satisfied and the set G is bounded.
then the Nemyckii operator F: X -+ X* is a monotone continuous potential
operator, i.e., there exists a convex C 1 .functional f: X -+ R such that
F = f'.
Moreover, F is maximal monotone, cyclic monotone, and hence 3*.monotone.
If(Al) and (A2) are satisfied and G is bounded, then the Nemyckii operator
F: X ..... X. is a continuous potential operator.
PROOF OF PROPOSITION 26.6. We set n = I, u = u., p = Pl. The deliberations
run analogously in the general case.
(I) Measurability. Since u e Lp{G the function x...... u(x) is measurable on
G, and, by (HI) and A 2 (12), the function
x  f{x, u(x»)
is measurable on G.
(II) F is bounded. Indeed. the estimate (7) follows from (H2) and A 2 (31).

564
26. Monotone Operators and Quasi- Linear Elliptic Differential Equations
(III) F is continuous from L,(G) into L 4 (G). To prove this, let
fI" -. u in Lp(G) as n -t> co.
By the convergence principle in Problem 26.4, there exists a subsequcnce
(u".) and a function v e Lp(G) with
UIJ'(x) -t> u(x) as n... 00 for almost all x e G
and the majorant condition
IfI".(x)1  vex) for all n' and almost all x E G.
Therefore, by the inequalities A 2 (30b) and (H2),
II Fu". - Full: = fG I/(x, u".(x» - I(x, u(x»1 4 dx
 const fG (1/(x. u".(X»14 + I/(x. U(X))!4) dx
::; const fG (la(x)14 + Iv(x)I" + lu(x)IP)dx.
By (HI),
I(x, u".(x» -- f(x, u(x» -t 0
as n -t 00
for almost all x e G.
JWajorized convergence A 2 (19) gives IIFu.., - Full., -.0, i.e.,
FfI". -. Fu in L,(G) as II -. 00.
The convergence principle (Proposition 1 O.13( 1» teUs us that the entire
.
sequcnce converges, I.C.,
Fu" -t> Fu in Lq(G)
as n -. 00.
o
.
The continuity of the Nemyckii operator F can also be proved in a simple
way by using the generalized principle of majorized convergence A 2 (19a)
instead of the convergence principle in (III) above.
The proof of Proposition 26.7 will be given in Problem 26.3.
26.4. Generalized Gradient Method for the Solution
of the Galerkin Equations
The Galerkin equations in Theorem 26.A represent a nonlinear system of
equations of the form
9,(X) = 0, x e A", i = 1, . . . , n. (8)
Let x = (1'. .., ,,) and)' = ('11'...' 'I,,). We investigate when (8) can be solved

26.4. GeneraJizcd Gradient Method for the Solution
565
\vith the aid of the iteration method
t+l) = 1t) - IYi(X(l:»,
for k = 0, 1. 2. . .. with x(O) = o.
i= I,...,n
(9)
Proposition 26.8. ASSllme that the !u,actions 9,: R" ..... R are continuorlsl)' differ-
entiable or at least locally Lipschitz continuous for all i. Srlppose tlaere exists a
number c > 0 sucl, that
ft ft
L (g(x) - g(.v»( - 'Ii)  c L (i - 'Ii)2
i1 i-I
for all x, Y E (R".
Then the s}'stem (8) has exactly one solutio" x E R", and the sequence (X(i)
constructed in (9) COli verges to x as k -.. 00 for sufficientl)' sn,all t > O.
This proposition is a special case of Theorem 26.8 below. In connection
with this theorem, we proceed from the operator equation
Gx = 0,
xeX,
(10)
with the iteration method
X i + 1 = Xi - tLGXt,
k = o. I, . . . ,
(11 )
where .'=0 = O. Our assumptions are the following:
(HI)  G: X ..... X are operators on the real H-space X.
(H2) G is strongly monotone and locally Lipschitz continuous. To be precise,
there exist positive numbers a and M(r) such that
(Gx - G}'lx - )')  all x - YII 2 for all X,}' e X
and
IIGx - Gyri s M(r)lIx - YII,
for all x, )' E X with nxll, IIYII s r and all r > O.
(H3) L is linear, continuous, self-adjoint, and strongly monotone, i.e.,
(Lxix) > bi l .xll 2
for all x E X and fixed b > O.
(H4) We assume the nontrivial case G(O) :F 0 and choose the numbers
c = liLli, ro = a- 1 (1 + #)IIG(O)H. to = 2afcM(ro)2.
Theorem 26.8 (Generalized Gradient Method). Ululer tile assumptiolJs (HI)
through (H4), the original equation (10) has exactly olae solrltion x E X.
If one chooses a fixed number t e ]0, t o [, the" tlae iterative sequence (x,) in
(11) cont"'erges in the H-space X to the solrltion x as k -+ 00. For all k = O. 1,
2, .. ., \ve IJave the error estimate
fix, - xII s a-1IIGxill.

566
26. Monotone Operators and Quasi- Linear Elliptic Differential Equations
Gradient methods will be considered in detail in Part III.
PROOF. We will use a majorant method. The basic idea of the proof is contained
in (14) below.
(I) Existence and uniqueness. We set X = X.. It follows from Theorem
26.A that equation (10) has a unique solution.
(II) Convergence of (x t ). Since the operator L is self-adjoint it follows from
b II y!J2  (L y I y)  c 1I} 11 2 for all y e X,
that hi S L S cl. This implies c- 1 1 < L -1 < b -11, i.e..
c -1 II y 11 2 S (L -I Y I y) s; b -In}t tl 2 for all }' e X
(cf. Problem 19.5g).
Let x be the solution of (10). i.e., Gx = O. Our proof of convergence
is based on the investigation of the functional
h(y) dcf (L -I(), - x)I} - x) for all ye X.
(II-I) By (11), for k = 0, I, ..., we have
hex,) - h(x t + 1 ) = 2t(GXt - Gxlxt - x) - t 2 (LGx"IGx,)
> 2talix" - xll 2 - t 2 cllGx t tl 2 . (12)
From (H2) and Gx = 0 it follows that allxll 2 S IIGx - G(O)R :lxII, there-
fore, we obtain the a priori estimate:
Itxtl S a-I It G(O) II  rOe
(11-2) Assume we know that
IIXtll  rOe
Then, from (H2) we obtain the estimate
IIGXA;II = IIGxt - Gxll S M(ro)lIx, - xII. (13)
(11-3) Let 0 < t < '0. We set
d = t(2a - tcM(ro)2
i.e., d > O. We want to show that, for all k = 1, 2, ..., we have the
following two ke}' estimates:
d IIxt.-1 - xl,1 S h(Xa:-l) - h(xa:),
IIx t - 1 11 :s; roe
\Ve prove this by induction. By (12) and (13), we have that (14) holds
fork = 1.
Suppose that (14) is valid up to a fixed k > I. Then
c-1Hxt - xII 2 S h(x t ) S h(Xt-1):S; ... S h(x o ) = h(O)
< b- 1 11x1I 2 S b- 1 a- 2 I1G(O)1I 2
(14)

26.S. Application to Quasi-Linear Elliptic Equations or Order 2m
567
and hence
IIXtll s IIXA; - xII + IIxll < roe
Moreover, it follows from (12) and (13) that
d HX t - 1I2  h(Xt) - h(x"+I).
(11-4) The key relation (14) tclls us that the sequence (h(x,» is monotone
itJcreasing and not negative, therefore convergent. Moreover, (14) shows
that .A: -+ X as k -t 00.
(III) The error estimate follows from Gx = 0 and (H2). This yields
a II x k - x II:Z  II G x A: - G x 1111 x A; - . H. 0
26.5. Application to Quasi-Linear Elliptic
Equations of Order 2m
As a first model equation we consider the boundary value problem
N
- L D.(ID i ul,-2 DiU) + SU = f on G.
;-1
(15)
U = 0 on oG.
Let G be a bounded region in R&v with N  1. Furthermore. let 2  p < 00,
p-l + q-l = l,and letsbea nonnegative real number. We set x = (I'...'"i)
and D f = a/Oi.
Definition 26.9. Let X = W p l(G). and let
a(r' v) = fG Ct ID,ul p - 2 DjuD, v + suv)dx,
b(v) = fG fvdx.
The generalized problem corresponding to (15) reads as follows: For given
f E Lq(G), we seek u e X such that
a(u,v)=b(v) forall veX. (16)
Formally, one obtains (16) by multiplying (15) by v e CO(G) and subsequent
integration by parts. The Galerkin equations corresponding to (16) read as
follows:
a(u", Wi) - b("i) = 0,
k = 1,..., n,
(17)
with
II
II" = L Ctll Wk.
'=1
In this connection, let {"'I' W2'...} be a basis in X. We set XII = span{ "'I'...' 'VII}.

568
26. Monotone Oratol'$ and Quasi-Linear Elliptic Differential Equations
Proposition 26.10 (Galerkin Method).
(a) The generaljzed problem (16) corresponding to (IS) has exactly one solution
u e X. The Galerkin eqllation (17) has exactly one solution u.. e X" .for each
n. and (rl n ) cont'erges in X to u as n .... 00.
(b) For s > 0 and fixed 'I, the solution U" of the Galerkin equation (17) call be
computed by the iteration method of Proposition 26.8 in the case ",here the
functions \V t and Di\va: are bounded OIl G for all i and k.
(c) The generalized problem (16) is equivalent to the operator equation
Au = b,
ue X,
",here (Au, v) = a(rl, v) for allll, veX, and the operator A: X -+ X. is
continrlorls. ulaiformlJ' nlonotone, coercive, and bounded.
PROOF. Ad(a), (c). It first follows from u e J-Jt,I(G) that DiU E Lp(G). Because
(p - l)q = p, we have
ID i ul,-2 D,u e L.(G).
By A z (4S), the embedding W,I(G) s L 2 (G) is continuous, i.e.,
lIull2 S consttlullx for all u e X.
According to A 2 (53b), we introduce the equivalent nor,n
lIuli = (fa I ID,uI P dx Y'P
on the Sobolev space X = w,t(G). This is an important step of our proof.
Let II. n. denote the corresponding norm on X..
(I) First key inequality. The Holder inequality along with (p - l)q = P
yields
la(u,v)1  f (fG IDfulPdx YI'I (fG IDjvl'dx YIP + sllullzllvlb
 const( n u 11'/4 + lIu II) I; vn for all II. v EX.
(II) Second key inequality. By (25.45)t
(IAI,-2l - Ipl p -2 p)(i. - Ii) > ciA, - IlI P .
for all  peR and fixed c > O. This implies
a(u, u - v) - a(v, u - v)  Clill - vII" + s fa (u - v)Z dx for allll, VEX.
(III) Equivalent operator equation. By (I) and (22.1 a), there exists an operator
A: X -+ X.
with
(Au, v) = a(u, v)
for all u, veX.

26.5. Application to Quasi-Linear Elliptic Equations of Order 2m
569
By (22.lb). we obtain bE X*. Thus the generalized problem (16) is
equivalent to the operator equation
Au = b,
UE X.
( 18)
We \vant to apply Theorem 26.A to this equation.
(IV) Properties of A. By (1),
fJAuli.  const(lIulI"q + lIull) ror all I' e X,
i.e., A is bounded. By (II
(Au - Av, II - v) > ellu - vll P for all u, v E X,
i.e., A is uniformly monotone, and hence A is coercive,
(V) Continuity of A via continuity of the Ncmyckii operator. In order to
prove the continuity of A: X -+ X. let
I'" -t> U in X
as II.... 00.
This implies
DiU" -t> DiU in L,,(G) as n.... 00,
according to the definition of the convergence in X = W p l(G). Set
F(u) = lul,-2 u ror all I' e R.
It follows from the gro\\1h condition
I F(I,)I < 1 ul p - t for all I' e R
and from Proposition 26.7 that the Nemyckii operator
F: Lp(G) -+ L.(G)
is continuous. Therefore, it follows from DiU" -. Du in L,(G) as n -II 00
that
f'(Dtu,,) -+ J:(D,u) in Lq(G) as n -. 00.
By the Holder inequality, for all VEX, we obtain that
I(AI'" - All,V)1 = J. L(F(Diu,,) - F(Diu)Div + s(u. - u)vdx
G i
S; L IIF{Diu,,) - F(D;u)II.,IID,vll p + sllu" - 111l211vllz.
i
From the continuity of the embedding X s L 2 (G) it follows that
I<Au.. - Au.v)1  const(  IIF(D,u.) - F(D,u)lI. + lIu.. - ull ) UvlI.
ror all VEX. This implies the key estimate
II Au" - Aull. < const (  IIF(D,14,,) - F(D,u)lI q + lIu.. - ull ),

570
26. Monotone Operators and Quasi-Linear Elliptic Differential Equations
and hence Ii Au" - Arlit. -. 0 as n ..... 00, i.e., the operator A: X ..... X. is
continuous.
(VI) Now it follows from Theorem 26.A that, for each be X, the operator
equation (18) has a unique solution U E X. Moreover, we obtain the
convergence of the Galerkin method.
Ad(b). We want to apply Proposition 26.8. To this end, we need the fol-
lo\\'ing inequality
11).1'-2 i. -lpl'-2 p l s (p - I)KP-2)).. - pi, (19)
for all A, It e R with IAI, 1#11 S K. Recall that p  2. In fact, in the case where
A, p  0, inequality (19) results from the mean value theorem of differential
calculus. In the case where Jl  0 < A. we use IlI P - 1 < (p - I)KP- 2 1,il.
We now set
gi(.) = a(u, Wi) - b(,,',)
with x = (1"".'1t) and
.
u = L rc 'Vie,
'=1
where  l' · · ., 'It are real numbers.
For all x, 'yeW, we obtain that
r. (g.Jx) - gt(Y»(t - 'It) ;:: S f. f «t - 'It)Wt)1 dx
tl G i.l
.
> C L (. - 'It)2,
'=1
where C denotes a positive constant. Moreover, the functions gi: Rit ..... R
are locally Lipschitz continuous. This follows from inequality (19) and the
boundedness of the functions 'Vt and D,WA; on G for all i, k.
The assertion (b) now follows from Proposition 26.8. 0
We now want to generalize Proposition 26.10 to quasi-linear elliptic equa-
tions of order 2m. To this end, we set
(Lu)(x) = > (-Icr1D' Acz(x, Du(x»
I '"
with m > 1. We consider the boundary value problem
Lu = f on G,
D' u = 0 on aG
(20)
for all fJ with IfJl S m - I,
(21)
where G is a bounded region in Di. tI with N > 1. We use the notation of
Definition 21.1. In (20) the summation is over all
 = (1'. · · , (IN) with II S nl,
where lal = c21 + ... + aN' i.e., the summation is over all partial derivatives DW

26.S. Application to Quasi-Linear Elliptic Equations of Order 2m
571
up to order m including u. In this connection, note our convention DOu = u.
We set
Du = (D1 U )hts.,
i.e., Du denotes the tuple of all partial derivatives up to order m including u.
The boundary condition in (21) means that all partial derivatives up to order
", - 1 should vanish on aGe
We now formulate several conditions on L. In this connection, we think of
A 2 as a real function of the variables x e G and D eRe", where
D = (DY)I>i"'.
(HI) Caratheodory cOIJditiolJ. For all (% with IIXI  m, the function A«:
G X RM -. R has the following two properties:
x...... A«(x, D) is measurable on G for aU D e RM;
D...... A«(x, D) is continuous on R M for almost all x E G.
For examplc, this condition is satisfied if A is continuous.
In the following conditions, let
1 < p < 00, p-I + q-I = 1, 9 e L 4 (G), he L.(G).
Moreover, let c, d, and C denote positive numbers and suppose that
the conditions are valid for all D, D' e (R.", lacl s m. and x e G.
(H2) Gro,vth condition
IA«(x,D)1 < C ( g(X) + L I D1Ip-I ) .
hi s-
(H3) Monotonicity condition
> (A(x, D) - A«(x, D'»(D 2 - D') > o.
rs.
(H4) Coerciveness condition
L A(x, D)D.  c > IDYlI' - hex).
1«1  1ft IJt='.
(HS*) Uniform monoton;cit}t
> (Acr(x, D) - A(x, D'»(D Gr - D'Gr)  d L IDY - D'YI'.
Js(S. hi-.
(H6.) Degeneracy. All the functions A« depend only on derivatives of u up to
order m - I.
A differential operator L in (20) is called a monotone coercive quasi-linear
elliptic differential operalor iff the conditions (H I) through (H4) are satisfied.
DefmilioD 26.11. Let X = W p l (G) with I < p < 00 and p-I + q-I = 1. The
generalized problem corresponding to the boundary value problem (21) reads

572
26. ionotone Operators and Quasi-Linear Elliptic Differential Equations
as follows. Let f E Lq(G) be given. We seck a function u e X such that
a(14, v) = b(v) for all t' EX. (22)
Here, for all u, t.' EX, \ve set
a(." v) = J. > AcI(x, Du(,,»DJt,(,,) dx,
Gtdrs",
b(v) = fG fvdx.
Formally, one obtains (22) by multiplying (21) by v E Co (G) and subsequent
integration by parts. The choice of the space X is meaningful for, it follo\vs
from" e J.J1 p "'(G) that [)Iu = 0 holds on aG, in the generalized sensc, for all {J
with IPI  nJ - I (cf. A 2 (48». Therefore, the boundary condition in (21) is valid
in the generali:ed sense.
Proposition 26.12 (Generalized Solutions of(21»). Assume (H 1), (H2), (H3), and
(H4). Then there e.,'-,;isIS exactl) one operator A: X --. X. such ,',at
(Au, v) = a(u, v) for all 14, VEX.
rhe generalized problena (22) correspondillg to (21) is equivalent to the operator
eq14atioll
Au = b,
U EX.
(23)
Tile operator A: X --+ X* is nJonotone, coercir..'e, continuous, and bounded. Thus,
(III the ilssertions of the mili" theorem on "IO'loto"e operalors (Theorem 26.A)
are valid for (23) and hence for (22).
III parliclliar. for each .f e Lf(G), tI,e ge"eralized problem (22) has a soilltion.
Corollary 26.13. If, in addition, co"dition (H5*) is I.,'alid, then the operator
A: X --+ X* is IIniJor"'I)' monotone. 1'1 this case, the solution u e X of (22) is
unique.
Corollary 26.14. Assume (HI), (H2), and (H6*). Then there exists e.actl}' one
operator A: X ...... X. such lltat
(Au, v) = a(r4, v)
The operator A ;s strongly continl4014s.
for all u, VEX.
Corollary 26.14 contains the observation, important for the next chapter,
that terms of lo\\'er order lead to strongly continuous operators.
PROOF. We use analogous arguments as in the proof of Proposition 26.10. For
brevity, the norm II' HIIt.p on the Sobolcv space X = "'(G) is denoted by II' II.

26.S. Application 10 Quasi-Linear Elliplic Equations of Order 2m
573
.
I.e. t
111111 = (f. L ID}'U(X)IPdX ) I/P.
G hi JII
The corresponding norm on X. is denoted by 11.11*. Recall that
lIull",.p.o = ( f. L ID 7 u(x)11' dX ) I!P.
c; h1 '!!! 8t
By A 2 (53b), the norm II. Ii m.p.O is equivalent to It .11 on X, i.e., there are positive
constants C 1 and C2 such that
c 1 11111i  IIlIlIm.p.o S c21111H for all II e X.
This relation will be used critically below in order to prove the coerciveness
and the uniform monotonicity of the operator A.
(I) The Ncmyckii operator. We set
(Fu)(x) = A(x. Du(.,)
Obviously, we have
D'/II e Lp(G) if u E W,"'(G) and 1)'1  nJ. (24)
Thus, it follows from (H I), (H2), and from Proposition 26.6 on the
Nemyckii operator that the operator
for all . e G.
Fm: X --. L.(G)
is continuous and
IIF«lIl1q < const(Ugl!. + Huf)"4) for all II e X.
(II) The key inequality. By the Holder inequality,
la(u, v)1  > IIfullfll1)«vllp
I III
< const(lIgli. + 1IlIlI,jq)fjvll for all u, VEX.
(III) Equivalent operator equation. By (II) and (22.la) there exists an opera-
tor A: X -+ X. such that
(Au. v) = a(u, v)
for all II, VEX
and
II Aull.  const( Ugll q + lIu IIP/4) for all II E X.
Hence A is bounded. Let f e Icl4(G). By the Holder inequality,
Ib(v)1  II /11'1 11 vII for all VEX.
Hence b EX..
ConsequentlYt problem (22) is equivalent to the operator equation
Au = b, II E X. This is (23).

574
26. Monotone Operators and Quasi-Unear Elliptic Differential Equations
(IV) Properties of A. By (H3), for all u, veX,
(All - AVtll - v) = a(u,u - v) - a(v,u - v)  0,
i.e., A is monotone.
By (H4), for all II e X,
(Au,u) = a(u,u)  cllull:'. p . o - fG h(x)dx
 ccfHuU" - const.
This implies (AUt u)/llull -. + 00 as lIu II ..... 00, i.e., A is coercive. Note that
p > I.
(V) Continuity of A. Let
u,,-.u in X as n-..oo.
Since F rI : X -+ Lf(G) is continuous,
Fu" ..... Fmu in Lq(G) as n -+ 00.
By the Holder inequality,
la(u", v) - a(u, v)1 S L II Fu" - F.ullqllvll,
S.
for all VEX. This implies
IIAu" - Aull.  > II Fu" - Fsrullq,
p...
and hence IIAu" - Aull* -+ 0 as n -+ 00. Thus, the operator A: X -+ X.
is continuous. 0
PROOF OF COROLLARY 26. J 3. By (H 5.), for all u, veX, we obtain that
(Au - Av, u - v) = a(u, u - v) - a(v, U - v)  dllu - vll.p.o  dcfllu - vll P ,
i.c., A: X -.. X. is uniformly monotone. 0
PROOF OF COROLLARY 26.14. By A 2 (45), the embedding operator correspond-
ing to
J-Jl p .{ G) S J-Jl p . -1 ( G)
is linear and compact, therefore strongly continuous. Let
U".....Ja..u in X as n -+ 00.
Since X = J-JlpJlt( G), this implies
u. ..... u in W p "'- I (G).
By assumption (H6*), the function A4I depends only on the partial derivatives
of u up to order m - I. Thus, as in the proof of Proposition 26.12 above,

26.5. Application to Quasi-Linear Elliptic Equations of Order 2m
575
\\'e obtain
II F(Arl" - F,ull q -. 0 as" -. 00
and hence Ii Au.. - AIII1. --. 0 as n -. 00, i.e.,
Au"  All in X. as n  00.
Consequently, the operator A: X -+ X* is strongly continuous.
o
Using the Sobolev embedding theorems, we want to relax the growth
condition,
(H2*) Relaxed gro\\,tll condition. For all x e G, D e RM, 1«1  m, and fixed
C > 0, we suppose that
IA(J(x, D)I  C ( g(X) + , IDi'IP1\(f. ) .
h.
y,'here 9 e Lq.(G) is given. Here, we choose the numbers P2' q« e ] 1, oc.[
in such a way that
1 m - 1«1 I
-- -
p  p
and p;1 + q;1 = 1. Rccall that m denotes the order of the differential
operator and N denotes the dimension of the bounded region G.
(H2**) Assume (H2*), \\'here "" in (25) is replaced by "< '.,
(25)
Proposition 26.1 S. A II the assert iOlls of Proposi' ;0" 26,12 (Ind Corollary 26.13
rer1w;n valid if \ve replace tile gro\,tIJ co,ulition (H2) by (H2*).
Corollary 26.14 remclins t'alid if \ve replace (H2) by (H2**).
Applications of this result will be considered in Section 27.4.
PROO.O, By A:z(45), it follows from (25) that the embedding
Wp.(G) 5 U1(G) (26)
is continuous. Hence we can replace (24) above by the following more general
rela tion:
DtJ II e L,.(G) if II e Wp.(G) and II < nl.
In the case (H2..) the embedding (26) is compact for II < nJ.
Now, the proof proceeds as the corresponding proofs of Proposition 26.12
and Corollaries 26,13 and 26.14 above. In this connection, we make essential
use of the continuity of the Ncmyckii operator described in Proposition
26.6. 0
Using more sophisticated arguments (e.g., the Holder inequality for several
factors together with the Gagliardo- Nirenberg interpolation inequalitics) it

576
26. Monotone Operators and Quasi-Linear Elliptic Differential Equations
is also possible to prove the continuity or the strong continuity of the operator
A: X -+ X. under much weaker assumptions than those made above. In
Proposition 27.11 we will consider a typical example in this direction, \vhich
is closely related to our existence proofs for the Navicr-Stokcs equations
(viscous now) and the von Karman plate equations in Part IV.
26.6. Proper Monotone Operators and Proper
Quasi- Linear Elliptic Differential Operators
A map is called proper iff the preimages of compact sets are again compact.
As we have seen in Section 4.14, the notion of proper maps plays a funda-
mental role in the study of the global properties of the solution set of the
operator equation
Au = b

u eX.
Further important results in this direction will be considered in Section 29.10.
The following proposition shows that important classes of monotone-like
operators arc indeed proper.
Proposition 26.16. Let AI' A 2 : X -. X. be operators on the real reflexive
B-space X. Set A = Al + A:z, and suppose that:
(i) Al ;s I,niforlnly monotone and cOIJlinuous.
(ii) A 2 is conlpact (e.g., strongly cont;nuol's).
(iii) A;s coercive.
TheIl, the operator A: X -+ X. is proper. Moreo'er, if A 2 is strongly con-
tinuous, tl,en A is pseudomOIJOlolle.
PROOF. By Theorem 26.A, the inverse operator A.l: X. -+ X is continuous.
Hence A 1 is proper by Section 4.14.
We want to prove that A is proper. To this end, let C be a compact subset
of X... We have to show that the set A -I (C) is compact. In fact, let (u lI ) be a
sequence in A- 1 (C). We set
b. = Atu ll + A 2 u".
Since C is compact, there exists a subsequence, again denoted by (b,,), such that
b ll -. b
as 11.... 00.
Since C is bounded and A is coercive, the sequence (u II ) is bounded. From the
compactness of A 2 it follows that there exists a subsequence, again denoted
by (rl,,)t such that
A 2". ..... C
as n -. 00.

Problems
577
Hence u" = AI1(b" - Azu,,) converges. i.e..
u..  u
as n -+ 00,
where u = Ai"I(b - c).
This implies A 1 U + A 2 U = b, since A 1 and A 2 are continuous. Hence II E
A -1 (C), i.e., .it -1 (C) is compact.
The pseudomonotonicit}' of A follows from Proposition 27.6 below. 0
As in Section 26.S, we consider the differential operator (20), i.e., we set
(Lu)(x) = L (- 1 )JI D A.(x, Du(x»,
12j s.
and we assume the following.
(A I) The Carathcodory condition (H I), the relaxed gro\\'th condition (H2*).
and the coerciveness condition (H4) from Section 26.5 are satisfied.
(A2) The highest-order terms of Lsatisfy the uniform monotonicity condition
(HS*), i.e., there is a number d > 0 such that
L (A 2 (x, D) - Acz(x, D'»(lY' - D''')  d L ID' - D'«I',
I-'" 1«1-"'
for all x e G and D. /Y e RM.
(A3) All the terms As \\'ith lexl < m depend only on derivatives ofr4 up to order
m - I.
We set X = Wp"'(G) and consider the operator A: X -+ X. which corre-
sponds to L, according to Proposition 26.12.
Proposition 26.17. Assume (AI) through (A3). Then the operator A: X -+ X. is
proper and pselldonwoolone.
PROOF. According to Propositions 26.12 and 26.15, there exist operators A I'
Az: X  X. such that
<A 1 U, v) = f. > A 2 (x, Du(x»DtJv(x)dx,
G Icz1=...
<A2'1, v) = f. L A«(x, Du(x»D 2 v(x) dx_
G 1«1 < ·
for all u. veX, where A = A I + A 2' and A I and A 2 satisfy the assumptions
of Proposition 26.16.
Thus, Proposition 26.17 is a consequence of Proposition 26.16. 0
PROBLE1tIS
26.1. Proof of Propos;' ion 26.2.
Solution: Let A: X  Y be strongly continuous. where X and Yare B-spaccs
and X is renexive. We want to show that A is compact. To this end, let (u.) be a

578
26. Monotone Operators and Quasi-Linear Elliptic Differential Equations
bounded sequence in X. Since X is reflexive. there exists a weak I)' con\'ergent
subsequence U.'  U as n  'XJ. Hence AU"I -+ All as n  00. Thus. A is compact.
Proposition 26.2(b) follows from Proposition 21.29.
26.2. Proof of Proposition 26.3, Ad(a). Let A: X  Y be demicontinuous. If A is not
locally bounded, then there exists a point u e X and a sequence u"  u as n -+ a)
with iIA"...... ex) as n -+ 00. Since A is demicontinuous, AII. Au as n -. 00.
Therefore. the sequence (Au,,) is bounded in contradiction to ' AU"II ..... 00 as
n -+ rJ).
Ad(b). Let A: X  Y be demicontinuous.lf A is not continuous from X (norm
topology) to Y (weak topology). then there cxists a point U E X, a neighborhood
V(Au) of Au in the weak topology of Y. and a sequence u" -+ u as n -+  such
that Au" f V(Au) for all n. This is in contradiction to All"  Au as n -+ OC'.
Conversely. if A is continuous from X (norm topology) to Y (weak topology).
then A: X -+ Y is demicontinuous. since continuity implies sequential continuity,
byA.(17e).
26.3. Proof of Proposition 26.7.
Solution: Let X = L,(G) with I < p < 00 and p-l + q-l = I. Then X. =-
L,(G). We set
(Fu)(x) a= f(x, u(.)).
By Proposition 26.6, the Nemyckii operator F: X ... X. is continuous and
bounded. If II. veX. then FII E X. and hence
(Fu,v)x = t f(x.u(x»v(x)dx.
Let ,f be monotone with respect to II. Then
[f(.t, u) - f(x, v)] (u - v)  0
for all u. v e R. x e G.
This implies
(Fu - Fv. u - v)x = L [f(x.u(x)) - f(x.v(x))](u(x) - v(x))dx  O.
for all u. veX. i.e.. the operator F: X  X. is monotonc.
let f be strictly monotone with respect to u. Then
(Fu - Fv, u - v).\' E: 0
implies
[f(x, u(x») - f(x, v(x»] (u(x) - v(x» = 0
for almost all x e G and hence u(.'t) = vex) for almost all x E G. This means u = t'
in X. Consequently, F is strictly monotone.
From f(x, u)u  dl u I P + g(x) it follo\\'s that
(Fu.u)x  d f,u,PdX + f gdx  dllull + f gdx.
This implies (Fu. u)/llull -+ +00 as lIuli -+ 00, i.e.. F is coercive.
Suppose that there exists an R > 0 such that
f(x, u)u  0

References to the Literature
579
for all (u, x) e G x R with lul  R. Let
A = {x e r: lu(x)1  R}.
Then the growth condition If(x.u)1 s C(a + blul,-I) yields
(Fu,u)x 2: J f(;t,u)udx + f f(x,u)udx
G-A A
 J f(x,u)udx  - r C(aR + bR")dx = -c,
G-A JG
for all u e X. If me as G < 00, then c < 00.
The more difficult proof of Proposition 26.7(e) concerning the condition (S)...
can be found in Browder (1971). p. 431.
26.4. A conLrgence theorem. Let 1 :s; p < 00, and let G be a nonempty measurable set
in R N , N  I. Show that if
u" --. u in Lp(G) as n -+ cx;,
then there exists a subsequence (un') and a function v e Lp(G) with
u..(x) -+ u(x) as n -. 00 for almost all x e G
and
lu..(x)1 S v(x)
for almost all x e G and all n.
Hint: This assertion results as a by-product in the proof of the completeness
of L,(G). Cf. Kufner, John, and FuCik (1977. M p. 74.
References to the Literature
Classical works: Viiik (1961 (1963) (partial differential equations), Minty (1962)
(1963) and Browder (1963 (1963a) (functional analytic theory).
Monographs on the theory of monotone operators: Browder ( 1968/76), Lions ( 1969)
(application to partial differential equations), Vainbcrg (1972) (connection with varia-
tional methods and Hammerstein integral equations), Brezis (1973) (maximal mono-
tone operators and time-dependent problems), Skrypnik (1973), (1986) (mapping
degree and elliptic differential equations), Gajewsk Grager and Zacharias (1974),
Langenbach (1976) (monotone potential operators), Barbu (1976, M) (time-dependent
problems), Pascali and Sburlan (1978 Kluge (1979 Deimling (1985).
Application to quasi-linear elliptic ditTerential equations: Lions (1969, M Browder
(1970, S Dubinskii (1976, S), Fucik and Kufner (1980, M), Petryshyn (1980a), (1981),
Nes (1983, M), Skrypnik (1986, M). cr. also the References to the Literature for
Chapter 27.
Nemyckii operator: Krasnoselskii (1956, M), Vainberg (1956, M), Browder (1971),
Pascali and Sburlan (1978, M), Appell (1987, S, B).
Numerical methods: Cr. the References to the Literature for Chapter 35.
History of the theory of monotone operators: Petryshyn (1970, S). Vainberg (1972, M
Brezis (1973, M), Dubinskii (1976, S
Recent trends: Browder (1986, P).

CHAPTER 27
Pseudomonotone Operators and Quasi-
Linear Elliptic Differential Equations
The classical development of nonlinear functional analysis arose con-
temporaneousl)' with the beginnings of linear functional analysis at about the
beginning of the twentieth century in the work of such men as Picard, S.
Bernstein, Ljapunov, E. Schmidt. and Lichtenstein, and "'as motivated by the
desire to study the existence and properties of boundary value problems for
nonlinear partial differential equations. Its most classical tool "'as the Picard
contraction principle (put in its sharpest form by Banach in his thesis of 1920-
the Banach fixed-point theorem).
Beyond the early development of bifurcation theory by Ljapunov and E.
Schmidt around 1905, the second, and eyen more fruitful. branch of the classical
methods in nonlinear functional analysis was developed in the theory of compact
nonlinear mappings in Banach spaces in the late 1920's and early 1930's. These
included Schaudcr.s well-known fixed-point theorem and the extension of the
Brouwer topological degree by Lcray and Sc:hauder in 1934 to mappings in
Banach spaces of the form I + C with C compact (as well as interesting related
results of Caccioppoli on nonlinear Fredholm mappings),
The central role of compact mappings in this phase of the development of
nonlinear functional analysis was duc in part to the nature of the technical
apparatus being developed, but also in part to a not always fruitful tendency to
see the theory of integral equations as the predestined domain of application of
the theory to be developed. Since, however. the morc significant analytical
problems lie in the somewhat different domain of boundary value problems for
partial differential equations, and since the effons to apply the theory of compact
operators (and in particular the Leray- Schauder theory) to the lattcr problems
have given rise to demands for ever more inaccessible (and sometimes, invalid)
a priori estimates in these problems; the hope of applying nonlinear functional
analysis to problems of this type centers on a general program of creating new
theories for significant classes of noncompacl nonlinear operators. The focus of
this study is then to find such classes of operators which have the opposed
characteristics of being narrow enough to have a significant structure of results
while also being wide enough to have a significant variety of applications... .
580

27. Pscudomonotone Operators and Quasi-linear Elliptic Differential Equations 581
From the point of view of applications to partial differential equations, the
most important class is that of monotonc-like operators.
Felix E. Browder (1968)
The first substantial results concerning monotone operators were obtained
b)' G. Mint)' (1962 (1963) and F. E. Browder (1963). Then the properties of
monotone operators were studied systematically by F. E. Bro\\'der in order to
obtain existence theorems for quasi-linear elliptic and parabolic partial differen-
tial equations. The existence theorems ofF. E. Browder were generalized to more
gencral classes of quasi-linear elliptic differential equations by J. Leray and J. L.
Lions (1965), and P. Hartman and G. Stampacchia (1966).
In this paper y,'c introduce two vast classes of operator namely, operators
of type (J\-f) and pscudomonotone operators. These classes of operators contain
many of those monotonc-like operators which were used by the authors men-
tioned abovc.
Ha.im BrCzis (1968)
Many problems in analysis reduce to solving an equation of the form
Au = b,
U E D(A),
where A is an operator on a space into another space. In this paper we assume
that A is a map on a subset D(A) of a Banach space X into another Banach space
Y + , \\'here {1': y 4} is a duaJ pair or Banach spaces. In an ideal situation. our
equation will have a solution u for every beY.. There is a large literature on
the "surjectivity" of this kind, including those related to monotone operators
and their generalil.8tions.
In the prnt paper we want to generalize the problem and seek sufficient
conditions for our equation to have a solution u for all "sufficiently smaJl h.'"
Our theorem. together with its companion for evolution equations, J have been
found useful in applications to man)' nonlinear partial differential equations.
Tosio Kato (1984)
In this chapter our goal is to treat, in contrast to Section 26.S, more general
quasi-linear elliptic equations having terms of lower order which satisfy no
monotonicity condition. We introduce pseudomonotone operators for this
purpose, i.e., we study the solvability of operator equations of the form
(E) A 1 U + A 2 U = b, u eX,
where Al + A 2 : X -. X. is a pseudomonotone and coercive operator on the
real reflexive a-space X. The prototype of a pseudomonotone operator is the
sum operator AJ + Az, where
(i) the operator AI: X -. X* is monotone and hemicontinuous; and
(ii) the operator A 2 : X  X. is strongly continuous.
Hence we obtain:
The ,',eOT}' of pseudomonOlone operators unifies both monoton;Cil} argu-
ments and compactness argumellts.
I See Theorems 27.8 and 30.8.

582 27. Pscudomonotone Operators and Quasi-Linear Elliptic Differential Equations
In connection with quasi-linear differential operators we use the following
important principle:
Lo\ver order terms correspond frequently to strongl}' continuous operators.
As we will show in Section 27.4, this is a consequence of the Sobolev embed-
ding theorems (compact embeddings). In order to transform quasi-linear
elliptic boundary value problems to the operator equation (E) above and to
be able to apply our general existence theorem for pseudomonotone operators
(Theorem 27.A), we need the following structure of the differential operator:
(a) The principal part of the differential operator is a monotone quasi-linear
elliptic differential operator in the sense of Section 26.5 (e.g., a linear
strongly elliptic operator).
(b) Thcre are nonlinear lower order terms which need not be monotonc.
(c) The differential operator is coercive.
This way we obtain the operator equation (E), where the monotone and
hemicontinuous operator A 1 corresponds to (a) and the strongly continuous
operator A 2 corresponds to (b). Condition (c) ensures that the sum AI + A 2
is coercive. This restricts the structure of the lower order terms.
In Part IV we will consider the following applications of the theory of
pseudomonotone operators to interesting problems in mathematical physics:
(a) The nonlinear \'on Karman plate equations in elasticity (Chapter 65).
({J) The Navier-Stokcs equations for viscous fluids (Chapter 72).
The proof of the main theorem on monotone operators in Section 26.2 was
based on the Galerkin method and the monotonicity trick. An inspection of
this proof shows that exactly the same proof works for more general classes
of operators than monotone operators. This is the basic idea of this chapter.
To this end, we introduce the conditions (M) and (8) as well as pseudo-
monotone operators. The condition (S) will also play an imponant role in
Part III in connection with variational problems and cigenvalue problems.
Pseudomonotone operators have the following nice property:
The stroltgl}' contiIJuous pertr,rbation of a pseudomonotone operator is again
a pseudomonotone operator.
The precise formulation may be found in Proposition 27.7. In Figure 27.1
in Section 27.5 we present graphically a series of important interrelations
between operator properties. We recommend a study of this diagram.
The main results of this chapter are the following:
(A) The main theorem on pseudomonotone operators of Brezis (1968)
(Theorem 27.A).
(8) The main theorem for locally coercive operators of Kato (1984) (Theorem
27.8).

27.1 The Conditions (M) and ( Convergcnce or the Galcrkin Method
583
This latter theorem is closely related to the main theorem for the semi-
coercive evolution equations of Kato and Lai (1984) (Theorem 30. B). Both
Theorems 27.8 and 30.8 represent an important recent technique in order to
solve nonlinear partial differential equations of time-independent as well as
of time-dependent type.
27.1. The Conditions (M) and (5), and the
Convergence of the Galerkin Method
DermitioD 27.1. Let X be a real reflexive 8-space. The operator A: X -. X.
satisfies the conditions (kl), (S)., (8), (S)o and (S). iff, as n -. 00. the follo\ving
hold.
(i) C ondilion (J\f):
14"  u,
A 14"  b.
Iim (Au",U,,)  (b,u)
" -. cc
implies
(ii) Condition (5)+:
Au = b.
u.u,
lim <Au" - Au, U II - ,,)  0
,,
implies
u,. -+ u.
(iii) Condition (8):
u. -:a. U.
lim <Au,. - Au.u. - u) = 0
implies
",. -+ u.
" - «X>
(iv) Condition (S)o:
u.  "
A",.bt
lim (Au,., u,,) = <b,14)
,,-
implies
(v) C01Jdition (S)1 :
U II ... u.
UII  14,
Au. ..... b
implies
",. -+ u.
Obviously, the following holds:
(S). => (S) => (8)0 => (8)1'
(1)
i.e., if the operator A satisfies the condition (S)+, then A also satisfies condition
(S), etc.
We now introduce the protot}'pes for (M) and (S)..
EXAMPLE 27.2. The following hold for A: X -+ X. on the real reflexive B-space
X:

584 27. Pseudomonotonc Operators and Quasi-Linear Elliptic Differential Equations
(a) The operator A is monotone and hemicontinuous implies that A satisfies
( J\f).
(b) The operator A is uniformly monotone implies that A satisfies (S)+.
PROOF. Ad(a). This is the monotonicity trick (25.4).
Ad(b). It follows from
- -
o < lim a( lIu" - ull) lIu" - ull s lim (All" - Au, II" - u) < 0
" ... t7:'
.
that
lim a(lIu,. - ulI) lIuli - ull = 0:
.oo
therefore also lim"...'X' lIu" - ull = 0 because of the properties of a(.) in
Definition 25.2. 0
The importance of (M) and (8)+ consists in the fact that, roughly speakin&
these conditions are invariant with respect to compact perturbations. We
make this precise in the following example.
EXA1t'PLE 27.3. Let A, B: X  X. be operators on the real reflexive a-space
x. Then the following hold:
(8) The operator A satisfies (5). and B is strongly continuous or, more
generally, B is compact implies that A + B satisfies (S)+.
(b) The operator A satisfies (8) and B is strongly continuous implies that
A + B satisfies (S).
(c) The operator A satisfies (M) and B is strongly continuous implies that
A + B satisfies (M).
We deal with a simple proof in Problem 27.1. We now study the significance
of (M) and (S)o for the convergence of the Galerkin method
(Au. - b, 'Vt) = 0, "II EX", k = I,..., n (2)
with X" = span {"'I'. . . , 'V n } for the operator equation
All = b, u E X.
(3)
Proposition 27.4 (Convergence of the Galerkin Method). Assume:
(i) Let A: X -. X. be a bOllnded operator satisfying condition (JW) on the real,
separable, refle:<;II'e. and i,J/inite-dime,asional B-space X. Let b e X*.
(ii) Let {\\'1' W2'. . .} be a basis in X.
(iii) TIJere exist an R > 0 and an no slIch that, for each n > no lhe Galerkin
eqllaliolJ (2) lias a solution u" \v;th lIu.1I s; R.
Then:
(a) There exists a subsequence (u..) with 14 11 ,  U as n  cc such that u is a
solution of (3).

27.2. Pseudomonotonc Operators
585
(b) If eqllatiolJ (3) has a unique solution u, then u"..... u as '1 -t 00.
(c) If A satisJles the condition (S)o illstead of (M), and A is dem;continlloIlS,
then olle call replace ,,,eak cont'ergence in (a), (b) by strong cOllvergelJce.
PROOF. Ad(a). It follows from (2) that
(Au.. - h, v) -. 0 as n.... 00 (4)
for all v e span {"'I' ''''2''''}' The operator A is bounded. Therefore. together
"'ith (II,,). the sequence (All,,) is also bounded. Consequently,
Au b in X.
II
as II'-',
(5)
by Proposition 21.26(c), (f).
The sequence (14,,) is bounded in the reOexive 8-space X. Thus, there exists
a subsequence (u..) with
U ,u
n
as n -+ 00.
It follows from (2) that
(Au"., II",) = (b, u ll ') -+ (b. u) as n -+ cc.
Now condition (M) yields Au = b.
Ad(b). Use the convergence trick (25.14).
Ad(c). In the proof of (a) we found a subsequence (u,..) with
U.'U' Au".b, (Au".,un,)-.(b,u) as n-+oc.
It follows from (S)o that
Un' -+ U
as n -+ 00.
The demicontinuity of A yields
Au".  Au
as n -+ r:r:,
and hence Au = b.
If the equation Au = b has exactl)' one solution u e X. then it follows that
the total sequence converges, i.e..
u" -+ u
as n -+ 00,
by the convergence trick (25.14).
o
27.2. Pseudomonotone Operators
In order to be able to apply Proposition 21.4 to a comprehensive class of
operators, we make available the following definition.
Definition 27.5. Let A: X ...... X* be an operator on the real reflexive B-space X.

586 27. Pscudomonotone Operators and Quasi-Linear Elliptic DilTerential Equations
(i) The operator A is called pselldo"wlwtone iff II,.  U as " -. 00 and
Jim (AU",fl" - u)  0
" .. oc
implies
(Au, u - w)  lim (A 14", U,. - w)
for all '''I eX.
,,-.
(ii) The operator A satisfies the condition (P) iff u"  u as n -. 00 implies
lim (All", U,. - U)  O.
....
We first give some prototypes.
Proposition 27.6. Let A, B: X -. X. be operators on the real reflexive B-space
X. Tlrell:
(a) If A is monotone and hemicontinuous, thell A ;s pseudomonotone.
(b) If A ia strongl}' contilluoUS, then A is pseudomonotone.
(c) If A is denl;colltinuous ,vith (S)+, then A is pselldolnOlloto'le.
(d) If A is continuous and dim X < 00, then A is pser4donw,wtone.
(e) Additivity. If A alld B are pseudomonolone thell A + B;s pseudomonotone.
(f) If A is nwnolone and hemicontinuous and B is strongl)' continuous, thell
A + B is pSelldolnonoto'le.
(g) If A ;s nlonotone and B ;s strongl}' continuous. then A + B satisfies (P).
Statement (f) plays a fundamental role in applications of the theory of
pseudomonotone operators to quasi-linear elliptic differential equations.
Obviously. property (f) is an immediate consequence of the additivity principle
(e) along with (a), (b).
PROOF. Ad(a). Let 14,,U as"  :() and
Iim (Au", u,. - u) < O.
.. -. 'XI
Since the operator A is monotone, we obtain that
(Au..,u" - u) > (AI4,u,. - u)-.O
as n.... 00.
This implies
(Au", u" - u) -. 0 as n -. 00,
since for this sequence. the upper and the lower limits are equal to zero.
Let z = u + 1('''. - u) \vith t > O. The monotonicity of A yields
(Au" - Az,u. - z)  0
and hence
,(Au",u - '''')  (-Au",u" - u) + (Az,u.. - u) + t(AZ,fl - ",).

27.2. Pscudomonotone Operators
587
This implies
(Az, u - \v)  lim (Au", u" - \v)
for all ", EX.
""00
The operator A is hemicontinuous. Thus, letting t -. Ot we obtain
(AI4, u - \\') < Jim (Au., u" - \\') for all \V E X,
""IX:
i.e., A is pseudomonotone.
Ad(b). If u.. 14 as n -. 00, then Au" -. Au, and hence
(All, U - \\1) = lim (Au", u" - \\1)
for all \" E X.
,,-oc
Ad (c). Let u,, u and Iim (Au", u. - u)  0 as n -. 00. This implies
lim (AI4" - Au, II" - u) S o.
II"
The operator A satisfies (S)+t and hence U lt ..... U as n -+ trJ. Since A is demi-
continuous. we obtain Au"  Au and hence
(Au, u - \v) = lim (Au","" - w)
for all", eX.
n"oo
Ad(d). This follows from (b). Note that weak convergence and strong
convergence coincide on Cinitc-dimensional B-spaces.
Ad(e). Let ""  14 as n ..... 00 and
Jim (Au. + Bu", u" - u)  O.
It-ex
This implies
lim (All", U II - II) S 0
and
fi -m (Bu., u" - u)  O.
(6)
. ... a)
" ... (X)
Otherwise, we may assume that there exists a subsequence, again denoted by
(u,,), such that
lim (Au.., u" - u) = a > 0,
" ... 
and hence Jim"...ctI (BII", u. - u) < -a. Since 8 is pseudomonotone, this
implies
(Bu, u - \\')  lim (8u lt , u. - w)
for aU \\'eX.
" ... 
Letting \\' = u, we obtain the contradiction 0 S - a.
Since A and 8 are pseudomonotone, it follows from (6) that, for all \\0' EX,
(Aut U - ",) S lim (Au", U II - w),
"...ct)
(But II - ",) < lim (Bu",Il" - w),
and hence
(Au + Bu, u - ",)  lim (Au ll + Bu., u" - ",)
" .. Q)
for all \" E X,
" -1]0
i.e., A + B is pseudomonotone.

588 27, Pseudomonolonc Operators and Quasi-Linear Elliptic Differential Equations
Ad(f). This follows from (a), (b), and (e).
Ad (g). The proof \vill be given in Problem 27.2.
o
Proposition 27.7 (Properties of Pseudomonotone Operators). Let A, B: X -+
X. be operlliors on the real reflexit'e B-space X. Then:
(a) If A ;.'i p.'ieudomonotone, then A satisfies the two condilions (P) a"d (,\11).
(b) If A ;s pseudomonotone and locall}' bOllnded, then A is demiconlinuous.
(c) If A is pseudomonotone and B is nWllotolJe and hemicont;Iulous Illen A + B
;.'i p.'iefldomonotone.
(d) If A is pseudonlonolone and B is strongly' conti,uIOIIS, tllen A + B ;s pseudo-
monotone.
Statements (c) and (d) represent perturbation principles for pseudomono-
tone operators, which follow immediately from Proposition 27.6(a), (b), (c).
PROOF. Ad(a). Let A be pseudomonotone. If A does not satisfy condition (P).
then there is a sequence (u,,) with
u u
"
as n -+ ::0
and
lim (Au", u" - II) < O.
"CC
From the pseudomonotonicity of A we obtain the contraction
o = (Au, u - u)  lim (Au", II" - ,,).
" .. ex:>
We shall now show that A satisfies (J\1). Let U"  U, Au"  b as n -+ 00 and
lim (Au'l'u,,) < (b,u).
"-00
Then limlJ (Au". u" - u)  O. The pseudomonotonicity of A yields
(AIl,u - \\') < lim (Au...u. - ",) fO'rall \tle X;
"-a)
therefore
(All. U - w)  (b. u) - <b, ",) = (b, u - ",)
Hence All = b.
Ad(b). Let (II,,) be a sequence with
for all \\' E X.
u"  u
as n  oc,.
Since A is locally bounded. the sequence (Au,,) is bounded. Let
Au.,b
as n -+ 00.
Then lim,,_1X> (Au"" II,,' - II) = O. The operator A is pseudomonotone;
therefore,
<Au. u - ",)  lim (Au".. u,,' - ",)
for all \V E X.
" - .x.

27.3. The Main Theorem on Pseudomonotone Operators
589
From this it follows that
(Au,u - ",) < (b.r4 - w) for all '" e X,
and hence AI4 = b. By the convergence principle (Proposition 21.23(i», the
total sequence (Au,,) is weakly convergent, i.e.,
Au h
"
as n --+ OCJ.
Thus, A is demicontinuous.
o
27.3. The Main Theorem on Pseudomonotone
Operators
We consider the operator equation
Au = b,
along with the Galerkin method
II e X,
(7)
(Au,. - b, \V Ie > = 0,
where XII = span {"'I'. · · , "',,}.
U II e XII'
k = 1,..., n,
(8)
Theorem 27.A (Brezis (1968». Assume:
(i) The operator A: X --+ X. is pseudonwnolone. bou,uled, a"d coercive 011 the
reat separable, and reflexif,'e B-space X \vith dim X = 00.
(ii) Let {",), "'2'. . .} be a basis ill X.
TIJell the .(ollo\\'ing hold:
(a) Existence. For each b eX., tIJe original equation (7) has a solution.
(b) Galerkin method. For fixed bE X. and for each n e N, the Galerkill
eql4atioll (8) has a solution u ll . TIJere exists a slIbseque'JCe (14",,) ,vhich con-
verge. weakl}' to a solution of the original equation (7).
If the operator A satisfies (S)..., tllen (u ll .) converges strony/} to a solut;on of
equation (7).
( equation (7) has a unique solution u, then tIJe total sequence (u,,) co,averges
to u.
PROOF. By Proposition 27.7, the operator A is demicontinuous and satisfies
(M).
As in the proof of Theorem 26.A, it follows that the Galerkin equation (8)
has a solution u", where III'" H < R Cor all n and fixed R > O. Now Proposition
27.4 yields the assertion. 0

590 27. Pstudomonotone Operators and Quasi-Linear Elliptic Differential Equations
27.4. Application to Quasi-Linear Elliptic
Differential Equations
We consider two simple examples which nonetheless illustrate two typical
situations. In both cases we have a monotone principal part. The lower order
terms yield strongly continuous perturbations. In particular, we shall show
how we can use the Sobolev embedding theorems in order to verify the strong
continuity.
We begin with the boundary value problem
N
- L D.(ID,ul,-2 DiU) + g(u) = f
'''1
on G,
u = 0 on oG.
Let G be a bounded region in IR N with N > 1. Let x ERA'. We set x =
(I ...  j'Y)' D i = iJ/Ch and we place the following conditions on the non-
linearity g.
(9)
(H 1) Coercit'elJess condition for g. The function g: R -+ R is continuous and
infllRg(U)U > -00.
(H2) Growth co,ulitio,J for g. For all u E iii,
Ig(u)1 s const( I + I Ul,-I),
where 1 < p q, r < 00, p-I + q-l = 1, and p-I - JV- 1 < ,-1.
In particular, we can choose r = p. In the special case N = 1, 2 and p > 2,
the number r can be chosen arbitrarily in the interval ]1, :X>[, since p-I -
N- I  o.
Instead of (9) we also consider the more general boundary value problem
N
- L D.(F.(Du» + g(u) = f on G,
i"l
(9*)
u = 0 on oG,
where Du = (D 1 U, . . . t DNu). In this connection, we make the following addi-
tional assumptions.
(H3) Monolonicity condition for the prilJcipal part. For all D D' ERN,
N
} (F.(D) - fi(D'»(D , - D;)  o.
t;1
(H4) Coerciveness condition for the principal part. There is a number c > 0
such that
A' A'
L F,(D)D, > C L ID,IP
1=1 1=1
for all DEAN.
(H5) Gro\\,th condition for the principal part. The functions F i : R'I -. Rare

27.4. Application to Quasi-Linear Elliptic Differential Equations
591
continuous for all i, and
I Ft(D)1 S const(l + IDI,-t) for all D eRr.' and all i.
Obviously, problem (9) is a special case of (9*) with
fi(D) = { ID i IP-2 D i f D, #:- O.
o If Dl = O.
Defmition 27.8. Let X = Wpl(G). The gelzeralized problem for (9*) reads as
follows. The function Ie Lf(G) is given. We seek 14 E X with
a 1 (14, v) + a:z(Il, v) = b(v) for all VEX. (10)
Here, for all u. veX, we set
J. ,'I
a 1 (u,v) = L fi(Du)Divdx,
G 1-1
a2(U.V) = fG g(u)vdx.
b(v) = fG fvdx.
Formally, problem (10) results from (9*) upon multiplication by ve CCf(G)
and subsequent integration by parts.
Proposition 27.9. Under assumptions (H I) through (H 5), the gelleralized problem
(10) correspo"ding to (9-) ',as a solurion u e X.
I" particular, under QSSIl,nptions (H 1) and (H2), the generalized problem Jar
(9) has a solutio" 14 EX.
PROOF. We will use Theorem 27.A and the results from Section 26.5.
(I) According to Proposition 26.12, there exists an operator AI: X  X.
with
a 1 (u,v) = (A1u,v)
for all 14, v EX.
The operator A 1 is monotone coercive, continuous, and bounded.
(II) According to Corollary 26.14 in the case where r = p, and according to
Proposition 26.15 in the general case, there exists an operator A 2: X 
X. with
a2(u,v) = (A 2 u,v) for all ll,veX.
The operator A 2 is strongly continuous.
(III) By (22.1b), bE X*. Thus, the generalized problem (10) is equivalent to
the operator equation
Al u + A 2 14 = b, u eX. (11)
We set A = Al + A 2'

592 27. udomonotonc Operators and Quasi-Linear Elliptic Differential F.quations
The operator A: X -. X. is continuous. By Proposition 27.6(f), the
operator A is pscudomonotone as a strongly continuous perturbation
of the continuous monotone operator A I'
The operator A 2 is bounded, since it is strongly continuous, and hence
A = A 1 + A 2 is also bounded.
It follows from assumption (H 1) that
(A 2 u.u) = fG g(u)udx  const forall UE X.
Hence A = AI + A 2 is coercive, since A I is coercive.
(IV) The main theorem on pseudomonotone operators (Theorem 27.A) yields
the assertion. 0
In the special case of problem (9), the operator AI: X -+ X* is uniforml)'
monotone, by Proposition 26.10. Thus, it follows from Examples 27.2(b) and
27.3(a) that the operator At + A 2 : X -t X. satisfies condition (5)..
Recall from the proof of Proposition 26.15 that the growth condition
Ig(u)1 s const(1 + lul,-I) is related to the compactness of the embedding
W p . (G)  L,( G).
Using our results from Section 26.5, it is possible to generalize Proposition
27.9 to quasi-linear elliptic differential equations of order 2m. A general result
about such equations will be considered in Problem 27.6. There we will use
the notion of sem;monotone operators, which arc special cases of pseudo-
monotone operators.
As a second simple but typical example, we consider in R'v with N = 1, 2,
3, parallel to (9), the boundary value problem
N
-L1u + 2 L (sin u)Diu = f on G,
1;1
(12)
u = 0 on oG,
where (% is a real number. 'rhe appearance of the first-order derivatives makes
it more difficult to prove that the lower order terms correspond to a strongly
continuous operator. To show this, we will use critically the compactlless of
the embedding
W 2 1(G) S L 4 (G)
in Rl\. with N = 1, 2, 3, and the Holder inequality for three factors. In Part IV
we will use the same technique in order to investigate the nonlinear von
Karman plate equations in elasticity and the Navier-Stokes equations for
viscous nuids. The coerciveness of (12) can be guaranteed if 1«1 is sufficiently
small. In this connection, we will use the Poincare-. Friedrichs inequality.
Definition 27.10. Let G be a bounded region in (RN with N = I. 2. 3, and let
X = Wl(G). The generalized problenl for (12) reads as follows: The function

27.4. AppJicalion to Quasi.Unear Elliptic Differential Equations
593
Ie L 2 (G) is given. \Ve seek u e X such that
Q. (II, v) + a2(u, v) = b(v) for all t: e X.
(13)
Here, \ve set
a.(II,v) = J f DjllDjvdx.
G i=l
J It
c(u, ,V, v) = (X r (sin u)(D, ,\,)t' dx,
G i:l
Q2(14, v) = c(u. u, v),
b(v) = fG fvdx.
Propositioo 27.11. There is a number o > 0 such that the generalized problem
(13) corresponding to (12) has a solution for all a: II  cXo and all f E L 2 (G).
PROOF.
Step I: Operator AI-
According to the proof of Proposition 26.10, there exists an operator
At: X -. X. with
(A.u,v) = a 1 (u,v)
for all u, veX.
The operator A. is uniformly monotone (and hence coercive), continuous, and
bounded. More precisely, the operator A I is linear, continuous, and strongly
monotone.
Step 2: Operator Az.
The Holder inequality yields
f (sin u)(D,u)vdx < (f (D j U)2 dX) 1/2 (f v 2 dx ) 112
and hence
laz(u, v)1 s const lIullxllvllx
for all II, VEX.
By (22.1 a), there exists an operator A 2: X -. X. such that
(A 2 u,v)=a 2 (u,v) for aU u,veX.
Thus, the generalized problem (13) is equivalent to the operator equation
AI u + A 2 U = b, u EX,
where b EX..
Step 3: Strong continuity of A 2 -
(I) By A 2 (45), the embedding X c L 4 (G) is compact
(11) Let u. u in X as n ..... CXJ. Then the sequence (u II ) is bounded in X. By (I),
U" -. U in L 4 (G)
as '1 -. 00.

594 27. P5eudomonotone Operators and Quasi-Linear Elliptic Differential Equations
We must verify
A 2 u" -+ A 2 11 in X.
as n.... 00,
.
I.e., as n -+ 00,
IIAzu" - A 2 ull = sup I(A 2 11" - A 2 11,v)I-+O.
)vlSI
Otherwise, there would exist an to > 0 and a sequence (v.,), which we denote
briefly by (v..), such that
IIv"lI x < 1
for all n
with
(A 2 11" - A 2 u, v,,)  £0 for all n. (14)
Passing to a subsequence if necessary. we can assume that v"  v in X and
hence
t'" -+ V in L.( G)
as n .... 00.
In order to obtain a contradiction, we use the decompositiolJ:
(sin II")(D;u,.)v,, - (sin U)(Dfll)V" = (sin u. - sin u)(Diu")v,,
+ (sin U)(Dill,,)(V. - v)
+ (sin U)(Dill" - DiU)V
+ (sin U)(Dill)(V - v.). (14*)
The decisive trick of our argument will be to use the weak convergence U"  II
in X with respect to the term (sin U)(Dill" - D;u)t\ From
Isin u.. - sin ul  I u" - ul
and the Holder inequality for three factors we obtain that
f (sin U" - sin u)(D,ulI)v. dx
 (f lUll - ul 4 dx Y14 (f I D{II" I 2 dx Yf2 (f I VII 1 4 dx y'
< II u" - 11114 [I u" II x II v" II x ·
By (14*), the same argument yields
I ( A 2 U,. - A 1 U. v" ) I  II U" - u 11 4 11 II" II x II v" II x + II u" II x II VII - V II.
+ c(u,"" - II, v) + II 1111 xII v" - vII. -+ 0
as n -+ co.
(14.*)
In rac \\'e have u" .... u and v" -+ V in L.(G) as II -+ 00, i.e., lIu" - 11114 .... 0 and
IIv" - vit 4 -+ o. Moreover the sequences (u.) and (v,,) are bounded in X.
Finally, we have
c(u u" - u, v) -. 0
as n -. 00.

27.S. Rclations Be('een Important Properties of Nonlinear Operators
595
This follows from "II " in X and
IC(Il, w, v)1 s const 1I\"lIx IIvllx
for all u, v, w eX,
according to the Holder inequality and hence the linear functional w.......
c(u, '''', v) is continuous on X.
Relation (14..) contradicts (14).
Step 4: Coerciveness of A 1 + A 2 .
ForallueX,
la2(u, u)1 < lal  J (sin u)CD,Il)u dx
 lal  (J (D,U)2 dx ) 112 (J 11 2 dx ) 1(2
 1(llliulii.
By the Poincare--Friedrichs inequality. there exists a c > 0 such that
a 1 (ll,u) > cllulli for all u EX.
This implies
(A 1 u + A 2 u,u) = QI(U,U) + a2(u,u)
 (c - 1(11) lIuli i for all u e X,
i.e., A 1 + A 1 is coercive if loci < c.
Step 5: Application of Theorem 27.A.
As in the proof of Proposition 27.9, we obtain that the operator A I + A 2
is pseudomonotone, continuous, and bounded. Now the assertion follows
from Theorem 27.A.
Moreover, AI + A 2 satisfies (S)+. 0
27.5. Relations Between Important Properties
of Nonlinear Operators
Proposition 27.12.// X and Y are real reflexive B-spaces, then the implicatiolas
in Figure 27.1 hold for operators A: X -. Y:
In this conlJeclion, let Y = X. in all the assertions that refer to monotonicilY,
pseudomonotonicity, hemicontinuity, coercivene.s, (M (S)., (5), (S)Ot (5)1' and
(P).
Explanation 27.13. In Figure 27.1 we only consider operators between real
reflexive B-spaces. The domain of definition is the total B-space. The arrows
are to be understood in the sense of an implication. The plus sign means the
operator sum, i.e. for example, (S)... + (compact) is the sum of an operator

596 27. Pscudomonotonc: Operators and Quasi-Linear Elliptic Differential Equations
-
-
-":
---

:::) u
 0 c
= 0
0 c -
- .- 0
0 - c
· C 0
C  8
0 e
E 0._ ==- 0
- e
 o  -0
5..c :s
 
C S
.-
-
.
-

-
.
tI:  
:s  ='
c 2 0
 - 
tI: C C C
 .-
0 - - -
c C c
 0 0 0
.:-. .S:! . .. .
- E E E
c
0  u u
u "'0 .: 
.
u
C
-0
-
o
C
o
E
o

=
S.
+
u
C
o
...
o
C .
o  c
S<===: 0 0
o - '-
-g 0 ;;
=' C ...
U 0
!. g
"'B
"'0
C

.8
;."
-
r:;  vs
... Cj = 
('2 0 0 0
u_   · -0
C C C -u u
.-
- - c-g
...  C
C C
84--8 0 
c 0
  N O
E - e >.
 .c 0= -
- .." U -g  I e.
- - 
c  vs
- c. .: r
--0 c .-
C U  ..J
8 /  u
_ C C
= U 0
e.& ""'='
>.c -
0
- = c
c . C . ]j : 0 .
.- 0 = S
c 2
='
:n

- 0 
u  u
C'2 C 
c- .- c
S -
c 
0  8..--. .
u
.. r ..:
... ...
 t'J t'J
U U U
C C C
.-
- - -
1
'-
-
-
('S
.- '"
- :s
 0
 
q-C
.=
_ c
8
 f1
-
0
=
C
.-
-
c
8
...


0-
S
0
v
-
'"
:s
o ...
::s ,.
,.. ::s
.: c
- :s
c C
0'-
u-
._ c
 0
- v


C
o
-
o c.
C E
o . 0
E.-
 -
- +
E
...

c


c
o
-
;-." 0 U
- C .,
co 0 'fj
S e--+
... 0
 v

C
o
...
o
;-"'c
-= 0
. e
'-
...

..
8-
o
'-
c.
I
u
C
Q :I)
- 
o 0
c 
o C
e .:;
c
- 8
e.e
 u
.- .:
C

-
-
v

..

-
-
r.I:,
=
o
::s
c
.-
-
c
o
'j
.-
- 
c
o
..
-
''>
-
+

--
o
-

-
!
-

-
..-.
M
-
r--=
f'1
C
o
.-
...

C
tU
C.
)(
L:.J
H
en

-
r-:
M

::s
CI.I


27.5. Relations Between Important ProJ>Cnics of Nonlinear Operators
597
\vith (S). and a compact operator. In particular, one infers from Figure 27.1
that every compact operator is bounded, and that monotone hemicontinuous
operators are pseudomonotone, etc.
Most of the assertions in Figure 27.1 have already been proved. We prove
the remaining assertions in Problem 27.3. In the following we summarize once
more all the important definitions concerning continuity and boundedness
properties of operators.
Definition 27.14. Let X, Y be B-spaces over IK = R, C. We define the following
properties for an operator A: X -+ Y:
A is contin"ous iff (u" -4 " implies Ar4" ..... Au).
A is strongl}' cOlltinuous iff (24"  u implies Au" -. Au).
A is demifont;IJUo14S iff (u"  14 implies Au"  Au).
A is \\'eakl}' sequeIJtial'>' cOlltinuous iff (r4"  u implies Au..  A 14).
A is cOlnpact iff A is continuous and maps bounded sets into relatively
compact sets.
A is il1,age cOl1'pact (i-compact) iff A is continuous and the range of A is
relatively compact.
A is proper iff the preimage of compact sets is again compact.
A is Lipschitz continuolls on M £ X iff
II Au - Avll  L Ilu - vII
for all U. I' E A-t and fixed L.
A is k-contract;ve iff A is Lipschitz continuous with L = k and 0  k < 1.
A is 1Jo1Jexpansif,'e iff A is Lipschitz continuous with L = 1.
A is locall}' Lipschitz cOlltinuous iff each U E X has a neighborhood V(II) on
\\'hich A is Lipschitz continuous.
A is uniform/}' continuous on M c X iff for each e > 0 there exists a b(e) > 0
such that, for u, ve J\I,
lIu - vII < b(t) implies !IAu - Al'lI < £.
A is bounded iff A maps bounded sets into bounded sets.
A is locall}' bounded iff each u E X has a neighborhood V(u) such that
A( V(u)) is bounded.
A is hemic01Jt;nuous in case Y = X. iff tt-+ (A(u + tv), \\') is continuous on
[0, I] for all u, v, \\' e X.
Note that u" -4 u stands for u" -+ u as n -+ cc, where n e N.
For mappings A: D(A) e X.... Y these definitions are to be modified in an
ob\'ious way. in that one considers only elements from D(A). One some-
times uses the term completely continuous for compact. Note, however, that
some authors understand a compact operator to be a continuous operator
\vhose range is relatively compact. We call such operators image compact
(i-compact).

598 27. Pseudomonotone Operators and Quasi-Linear Elliptic Differential Equation.
27.6. Dual Pairs of B-Spaces
In the preceding sections we considered operators of the form
A:X-.X.
from a 8-space X to its dual space X. .In order to be able to apply our abstract
results to more general partial differential equations, we now study operators
of the form
A: X -. X....
where {X, X+} is a dual pair of a-spaces.
Definition 27.15. Let X and X+ be B-spaces over ()( = R. C. Then {XX+} is
called a drlal pair iff the following hold:
(i) There exists a bilinear. bounded map
(v,u) -. (v,u)x
from X+ x X to IK.
(ii) If (v, u)x = 0 for all u e X, then v = o.
(iii) If (v. u)x = 0 for all v e X+. then II = O.
STANDARD EXAMPLE 27.16. Let X be a s.space X and let X. be its dual
space. Then {X. X.} is a dual pair if we set, in the usual way.
(v, u)x = v(u) for all veX., u e X.
EXAfPLE 27.17. Let X = C(G), where G denotes a bounded region in R N ,
N > I. If we set
(v.U)x= f, uvdx,
Ci
(I S)
then {X, X} is a dual pair.
Moreover, if we set X = W 2 "'(G), m = 0, I, .... and X" = L 2 (G), then
{ X. X +} forms a dual pair with respect to (I S).
In contrast to the Standard Example 27.16. in this example we do not have
X'" = X*. In fact, dual pairs are a useful generalization of the usual duality
for B-spaces.
27.7. The Main Theorem on Locally
Coercive Operators
We consider the operator equation
All = b,
u e D(A),
(16)

27.7. The Main Theorem on Locally Coercive Operators
599
with respect to the operator
A:D(A)X-+Y+,
(17)
\\'here
(K r\ Y)  D(A).
We are given b E X. \vith X+ S Y.. Our goal is Theorem 27.8 below. This
theorem allo\vs interesting applications to nonlinear partial differential equa-
tions. In Section 27.8 we will consider such an application. In this connection,
the local coerciveness condition (H3) below is t--ssential. Furthermore, it is a
typical pecular;t} of our situation that the operator A is not defined on the
total B-space X but only on a subset D(A) of X. In order to compensate for
this fact, we use '\\'0 B-spaces X and Y with
y X.
Roughly speaking, the space Y is "better" than the space X. For example, in
applications to differential equations, the space Y contains smoother functions
than the space X. The basic idea of our existence proof is to use a Galerkin
method in the "better" space Y which converges only in the "worse'. space X.
This idea is related to the basic idea of the hard implicit function theorem
in Chapter 5. There we constructed an iteration sequence (l'lt) with U lt E X. for
aliI! and
X e x c...eX
1- 2- - ,
where (u,,) converges in the .bad" space X.
The use of different spaces combined with convergence in "bad" spaces is
important for the modern theory of nonlinear partial differential equations.
In Chapter 30 we will apply this idea to evolution equations (Theorem 30.8).
In what follows the reader should think of the standard situation that X
and Y are B-spaces with the continuous and dense embedding
YX
(e.g., Y = X). This implies the continuous embedding
X* c Y.
in the sense that a linear continuous functional b: X  D< is also a linear
continuous functional of the form b: Y -. IK, by restricting b to 1': If we set
X. = X*.
Y'" = Y.,
then we obtain dual pairs {X, X+} and {Y: y+}. In this situation, the set K
can be chosen as a closed ball in X.
We make the following assumptions:
(H I) Dllal pairs. Let the dual pairs {X, X +} and { Y +} be given, where X,
X +, Y, y+ are real B-spaces with the corresponding bilinear forms
< · , . )x and <., · >r and the continuous embcddings
Y c X and X+ c y+.

600 27. Pseudomonotone Operators and Quasi-Linear Elliptic Differential Equations
The t\\'O dual pairs are compatible, i.e.,
(b, u)x = (b,ll>r
for all b e X+, U e 1':
Moreover, the B-spaces X and Yare separable and X is reflexive.
(1-12) Operator A. Let the operator A: D(A) c X --. Y+ be given, and let K be
a bounded closed convex set in X containing the zero point as an interior
point and K n Y c D(A).
(H3) Local coerc;velJess. There exists a number (J.  0 such that
(Av, v)r  a
for all v E Y f'e vK,
where oK denotes the boundary of K in the B-space X.
(H4) COlJtinuit)'. For each finite-dimensional subspace Yo of the B-space Y,
the mapping
\vt-+ (A"" v)r
is continuous on K n Yo for all v E Yo.
(HS) Generalized condition (J"'). Let (u,,) be a sequence in Y n K and let b E X + .
Then, from
U" .....2It. U in X
as n --. 00
( 18)
and from
(All", I' >r -+ (b, I' >r
as n... 00
for all v E Y,
and
lim (Au", Il,,)r  (b, u)x,
"  :()
it follows that Au = b.
(H6) Quasi-boundedlless. Let (u lI ) be a sequence in Y n K. Then, from (18) and
(Au n , u,,)r  const lIu"lI x for all n E N
it follows that the sequence (Au,,) is bounded in Y....
Note that the conditions (H4), (HS) and (H6) are satisfied if, for example,
D(A) = K and the operator A: K e X.... Y+ is weakly sequentially continuous.
(H7) Local strict monotonic;t}7. There exists a dual pair {Z, Z+}, \vhere Z and
Z+ are real B-spaces with the continuous embeddings
X £; Z and Y  c Z" ,
such that
(Au - Av, U - v)z > 0 for all II, v E D(A) ,,'ith u:# v.
(H8) Local strong InonotolJ;cit}'. In addition to (H7), there exists a number
d > 0 such that
(Au - At', U - v)z  dllu - vll
for all II.. (: e D(A).

27.7. The Main Theorem on Locall)' Coercivc Operators
601
Theorem 27.8 (Hess (1973), Kato (1984». Suppose that the assumptions (HI)
tllrollglJ (H6) are satisfied. Then the follo)'ing hold:
(a) Existence. For each b e X+ with
<b, v>x < a for all v e K tl 1':
equation (16) has a solutio" u, i.e., b E R(A).
(b) Uniqueness. This solution is unique if, in addition, condition (H7) holds.
(c) Continuous dependence of the solution on the data. 1/, in addition, condi-
tion (H8) holds, then il follo)\'5 from AUi = b l and hi E X+ for i = I, 2 tlJat
Ulll-u2I1zconstUbl-b2I1z..
This is a generalization of a related result in Kato (1984). OUf generalization
is useful with respect to the applications to strongly nonlinear differential
equations in Section 27.8.
Obviously, Theorem 27.8 is a generalization of the main theorem on
pseudomonotone operators (Theorem 27.A). In fact, Theorem 27.8 is a power-
ful tool for handling nonlinear partial differential equations.
Corolla!)' 27.18 (The Special Case of Balls). Suppose lllat conditions (HI)
tllrouglJ (H6) IIold. Let K O denote the polar set of K, i.e..
K O = {b e X+: (b,v>x  I for all v E K}.
TIJen a.Ko s; R(A), i.e., the original equation (16) has a sol14tion u for eaclJ
b e aeKO.
/'1 particular, if K is a ball of radius R > 0, i.e.,
K = {ve X: Jlvllx S R}
and if
< b, v> x Sell b I! x. II v II x
and fixed c > 0, then
for all beX+, ('eX,
{b e X+: IIbli x . S l/eR}  KO,
i.e., equation (16) has a solution u for each b e X. with IIbli x . s «feR.
This is an immediate consequence of Theorem 27.8.
Corollary 27.19 (Global Coerciveness). Suppose thai the conditions (H I)
through (H6) are valid for all balls K in X, and suppose that the global coercive-
ness condition
<Av, v)r/II vII x --. + cc as II vII x -+ 00, v e 1':
is satisfied. Then X. c R(A), i.e., equation (16) has a solution ufor eac/l b e X+.

602 27. Pscudomonotonc Operators and Quasi-Linear Elliptic Differential Equations
PROOF. This follows from Corollary 27.18. Notc that we can choose a = {l(R)R
with fJ(R)..... + as R -+ +00. Hence a./cR .... +00 as R  (fJ. 0
PROOF Of THEORM 27.B(a). We use a similar argumcnt as in the proof of
Theorem 27.A. More precisely. we consider a Galerkin method in the space
Y which converges in the space X.
If Y = {OJ, then Y. = to}, and the statement of Theorem 27.8(a) is trivial.
Therefore, let us suppose that Y #: to} and X #: to}.
Since Y is separable, there exists a sequence
Y 1 c: Yz c: ... c y
of nontrivial subspaces  of Y such that the set Un  is dense in  We set
K.. = K r\ Y n .
The embedding Y 5 X is continuous. This implies that each open (resp.
closed) set in X is also open (resp. closed) in y: Consequently, K. is a closed
convex subset of  containing the zero point as an interior point according
to (H2). Moreover, cK" 5 aK.
For each nonzero v e Y.., let
llvU = inf{i.. > 0: i.. -I V e oK n },
i.e., 11.11 is the Minkowski functional of K.. Therefore If. H is a norm on Y".
Since dim  < 00, the mapping u..-. lIuli is continuous on Y". Thus, we obtain
that
intKn = {VE Y n : IIvll < I}
and oK" = {v e Y,,: IIvll = I}.
Step I: The Galerkin equations in Y.
We consider the Galerkin equation
(Au.., v)r = (b. v)r for all v E Y.. (19)
We are given be X+ with (b,v>r < a for all v E K", and we are looking
for
Un e K..
By the local coerciveness condition (H3), we obtain the ke)t co,ulit;on
(Av, v)r - (b, v)r > 0 for all v e oK.. (20)
Step 2: Solution of the Galerkin equations by using the existence principle
in Section 2.4.
Let {"'I'.... ,":v} be a basis in . We set
N
x = L i"'i
i-I

27.7. The tain Theorem on Locally Coercivc Operators
603
and identify RaY with Y". Moreover, let
gi(.X) = (Ax, Wi)r - (b, \\'i )r.
Then the function g: K. c RaY -+ (RN is continuous, by (H4). From (20) it
follows that
.Y
L g.(X)i > 0
i1
for all x e iJK,.,
that is, for all ., e R- Y with II x II = 1. By Section 2.4, the system
gi(.) = 0,
X E K",
i= I,...,N,
has a solution, i.e.. the Galerkin equation (19) has a solution Iin e K".
Step 3: Convergence of the Galerkin method in X.
By (H2), the set K is bounded in X. Thus, the sequence (u II ) with U lt e K for
all " is bounded in the reflexive B-space X. Consequently, there exists a
subsequence, again denoted by (u,,), such that
UIIU in X
as n  00.
(21)
From the Galerkin equation (19) and (HI) it follows that
(Au", u.)r = (b, u,.)r = (b, u,.)x
and hence
(Au", u..)r S const IIbli x . Uu"lIx for all n.
By (H6), the sequence (Au,.) is bounded in Y..
Let v e U. Y", i.e., v e  for a fixed k. By (19),
(Au., v)r ..... (b, v)r as n -+ 00. (22)
Since the set U. f" is dense in Y and the sequence (Au,,) is bounded in Y+, the
relation (22) also holds true for all v e  This follows from a simple approxi-
mation argument. by using the fact that the bilinear form (\\', v)...... (\\1, v)r is
bounded from Y + X Y to IR.
From (19) and (21) we obtain
lim (Au",uII)r = lim (b,II..)x = (b,u)x.
""00
" .. aC l
By assumption (H5), Au = b.
PROOF OF THEOREM 27.B(b). From AU 1 = AII2 we obtain U 1 = U2 by (H7).
PROOF OF THEOREM 27.B(c). Condition (H8) implies
dUll! - ull1 S constllu 1 - u2l1zllAu, - Au 2 U Z .
and hence
lIu. - 14 2 11 z < constllAu , - AU2"Z..
o

604 27. Pscudomonotone Operators and Quasi-Linear Elliptic Differential Equations
27.8. Application to Strongly Nonlinear
Differen tial Equations
We consider the boundal)' value problem
- u + g(u) = f on G,
u = 0 on oG.
(23)
In contrast to Section 27.4, there are no growth conditions for g. There-
fore, we speak of a "strongly nonlinear" problem. For example, think of
g(r4) = eM.
We make the following assumptions:
(A I) The function g: IR -. R is continuous with (g(14) - a)14 > 0 for all u e R
and fixed real a.
For example, this condition is satisfied if g: R .... R is continuous and mono-
tone increasing (e.g., g(u) = elf). In this case, we choose a = y(O).
(A2) G is a bounded region in R N with piece\\'ise smooth boundary, i.e.,
aG e Co. 1 .
The basic idea of the following investigation of problem (23) is to reduce
(23) to an operator equation
Au = b, " E D(A),
where
D(A) = {14 e X: II E L.(G)}
and IJ(:() = (g(u(Jc» - a)r4(x), i.e., the operator A is not defined on the total
B-space
x = W 2 1 (G).
Moreover, we set
Y = W:(G) " X, k > Nj2,
and Ilulir = lIullk.2. Since k > Nj2, the embedding
y c: C(G)
is continuous by the Sobolev embedding theorems. Note that
Ig(u)1  (g(r4) - a)u + canst for all U E R,
.
since
Ig(u) - al = lu- 1 (g(u) - a)ul s (g(u) - a)u for lul > 1.
Therefore, from u e D(A), it follows that x...... g(u(,» belongs to L 1 (G).
Definition 27.20. The generalized problem corresponding to (23) reads as

27.8. Application to Strongly Nonlinear DifTerenlial Equations
60S
follo\vs. Let f E L 2 (G) be given. We seek II e D(A) such that
(J t (II, v) + a2(u, v) = b(v) for all v e Y:
(24)
where
f. .'1
at (u. I'> = > DuD,I,d..
G
Q2(U.V) = fG g(r.(x))v(x)dx.
bet') = fG Iv dx.
Formally, \\'C obtain (24) by multiplication of (23) with v e CO'(G) and
subsequent integration by parts. The ideci behind the construction of the set
D(A) is to guarantee the existence of a2(u, .,) and a2(II. u) for all u e D(A) and
ve Y.
Proposition 27.21. Suppose that (AI), (A2) Iwld. The", for eacll Ie L 1 (G), lite
bouIJdar}' value problem (23) has a generalized solut;oll U E D(A).
PROOF. Passing from (23) to
- Au + (g(u) - a) = f - at
we can assume that a = O.
We apply Theorem 27.8 to a sufficiently large ball K in the B-space X.
Moreover, we set
X*=X",
Y. = y...
The key to our proof will be (V) below.
The Holder inequality yields
Ib(v)1 S constllfll211vllx
forall veX.
Hence b EX..
(I) Operator A l' By the Holder inequality, we have
la1(u.v)1 s constlluilxHvllr for all ueX. VE Y.
Hcnce thcre exists exactly one linear continuous operator A I: X  Y.
with
(A 1 u,v)r = al(uv) forall UE X VE 1':
(II) Operator Az. Let II E D(A). Then
la2(u. v)1  fG Ig(u)1 dx IIvllc«11
 constllvlly for all ve 1':

606 27. Pscudomonotone Operators and Quasi-Linear Elliptic Differential Equations
Thus, there exists a uniquely determined operator A 2 : D(A) e X... Y.
with
(A 2 u,v)r = a z (lI,v) for all u e D(A), v E Y.
(III) Operator A. We define A: D(A) 5 X -. y* by
A = Al + A 2 .
Then, the generalized problem (24) corresponds to the operator equation
Au = b, U E D(A). (25)
Note that Y 5 C(G) S D(A)  X.
(IV) Global coerciveness of A. Noting g(lI)u > 0 on R and the inequality of
Poincare- Friedrichs, we obtain that there exists a constant d > 0 such
that
(Av, v)y = a l (v, v) + az(v, v) > a. (v, v)
 dllvlli for all v E y:
(V) Generalized condition (M). Let b E X. and let (u II ) be a sequence in Y with
UIIU in X
as n-.oo
and
(Au", v)y -. b(v) as n -. 00 for all v e Y, (26)
lim (Au,.. u,,)..  b(u). (27)
II ... QO
We want to show that this implies All = b.
In the following, c denotes an arbitrary constant. The operator A I: X -. Y.
is linear and continuous, therefore weakly sequentially continuous, i.e.,
(A III", v)t. -. (A I II, v)r
as n-.oo
for all v e y:
Because of (26) it is sufficient to prove that U E D(A) and that
(A 2 II", v)r -. (A 2 u, v)y
as n-.oo
for all v e y:
Noting Y!: C(G) and (A2UV>r = IGg(u)vdx along with the estimate (II), it
is sufficient to show that
g(II,,(X» -. g(u(x») in L 1 (G)
as n -. 00.
(28)
Moreover, note that it is sufficient to prove (28) for a subsequence of (u,,).
The following proof of the conditions U E D(A) and (28) is based on the
Lemma of Fatou and the Vitali convergence theorem.
(V-I) We show that (}(u(.))u(.) e L 1 (G), i.e., u e D(A). The embedding X c
L 2 (G) is compact. This implies
Un -. U in L 2 (G)
as n -. 00.

27.8. Application to Strongly Nonlinear Differential Equations
607
Thus, there exists a subsequence, again denoted by (u,,), such that
u,,(x) -+ u(x) as n.-. 00 for almost all x E G.
Moreover, from Un --:a. U in X it follows that
sup lIu.1I x < 00
"
and hence
(A 1 U", UII)r S ell u"lIi S coost.
Relation (27) yields
lim (A 2 u", u,,»), = lim f. g(ulI)u"dx  C, (29)
"-''7) "-'0) G
and the continuity of g implies
g(rl,,(..'(»rl,,(..'() -+ g(u(x»u(x) as'1 -+ 00 for almost all x E G.
Therefore, the Lemlna of f.alOU A z (19c) tells us that
g(u(" »11(") e L 1 (G).
(V-2) We prove (28). Let d > 0 be fixed. For each x e G, we have either
1 u,,(x)1  d
or
Ig(u,,(x»1  d -I g(u,,(x»U,.(x).
Note that g(u) = u- I g(u)u if U  O. Moreover, we get
(g(rl)1  c(d) for all II: lu 1 S d.
Let H be a measurable subset of G. Then,
f Ig(fln)ldx < c(d)measH + d- ' f g(u,,)"n dx
JH Ju
 c(d)measH + d-.c , .
By (29). the constant CI is independent of n, d and H. Hence
In Ig(u.)1 dx < e/2 for all n
if d is sufficiently large and meas H is sufficiently small. Noting the
absolute continuity A z (20) of the integral, we obtain the following. For
each t > 0, there exists a (£) > 0 such that
In Ig(",,) - g(u)1 dx S In (lg(".)1 + Ig(u)l) dx < t,
for all n and for all subsets H of G with meas H < b(l:).

608 27. Pseudomonotone Operators and Quasi-Linear Elliptic Differential Equations
Therefore, the Vitali convergence theorem A(21) tells us that (28)
holds true.
(VI) Quasi-boundedness of the operator A. Let (rl,,) be a sequence in the space
Y with
rl,,u in X
as II -+ XJ
and suppose that
(Au.., u,,)r  const II u" II x for all PI.
We want to sho\v that the sequence (Au") is bounded In Y*. The
boundcdncss of (rl,,) in X implies
lim (Au", u,,)r < const.
" ... I7J
In order to obtain a contradiction, suppose that (All,,) is unbounded
in Y*. Then there exists a subsequence, again denoted by (u II ), such that
II AuIIII f. -+ 00
as n -+ 00.
(30)
By the same argument as in (V) above, we obtain that
(Arl",v>r -. (Au,v)r
as n-+oo
for all v E Y,
by passing to a subsequence, if necessary. The uniform borlndedness
principle Al (35) tells us that the sequence (Au,,) is bounded. This con-
tradicts (30).
Now it follows from Corollary 27.19 that the equation Au = b, u E
D(A), has a solution if b E X*. 0
PROBLaIS
27.1. Proof of E.'tanJple 27.3.
Solution: Ad(a). Let
".  u.
Iim <Au. + BUrt - Au - Bu, Un - u) < 0
as n -+ .
Since (u.) is bounded in the reflexive B-space X and the operator B is compact,
there exists a subsequence (u..) such that BUll' -+ b as n -.. 00 and hence
lim <Au.. - Au, u.' - u) < O.
rt-x-
The operator A satisfies (S)+; therefore Ulf' -.. u as n -.. ex:;.
By the convergence principle (Proposition 10.13(1) the total sequence (u ll )
converges.. i.e Urt  U as II -.. 00.
Ad(b). Let
". -4 U,
<Au. + BUll - Au - Bu, U II - u) -+ 0
as n... OCt
The operator B is strongJ)' continuous; therefo BUll -+ 8u and hence
(Au. - Au.u. - u)-.O
as n -+ C£).
The operator A satisfies (5); consequently U II -t U as n  00.

Problems
609
Ad(c). Let C = A + 8, and let
u.u. Cu,,b. lim (Cu".u,,) S (b.u)
as n -+ oc.
The operator 8 is strongly continuous. i.e.. Bu"  Bu and hence
mn (Au..u,,) s (b - Bu,u).
" -. (It
The operator A satisfies (J\1). Thus. from Au"  b - Bu as n -+ oc it follows
that Au = b - Bu, i.e.. Cu = b.
27.2. Proof of Proposition 27.6(g). Let u"  u as n ... XJ. The operator 8 is strongly
continuous; consequently
lim (8u". u" - u) = O.
" ... OCt
The operator A is monotone, i.e.,
(Au.., un - u)  (Au, u" - u)  0
Hence lim "..oo (Au" + Bu", u" - u)  O.
27.3. Proof of the assertions ;11 Figure 27.1. Provc thc assenions that havc not yet
been proved in Chapters 26 and 27.
Let A: X ... Y be an operator, where X and Y are B-spaces o\'er K :: R, C.
(I) Show that if the operator A is strongly continuous. then A is uniformly
continuous on bounded sets in the case where X is reflexi\'e.
Otherwise, there exist an 1.:0 > 0 and bounded sequences (un), (V,,) with
Ilu" - v,,11  l/n and
as n  .
II Au" - At:,,11 > to
for all n EN.
Because of the reflcxivity of X there then exist subsequences U,,'  u and
V..'-' v as n ... : therefore, u  v and Au", - Av". -t 0 as n -t . This is
a contradiction.
(II) Show that if the operator A is uniformly continuous on bounded sets.
then A is bounded.
I..et M = {u e X: lIuli s r}. The operator A is uniformly continuous
on M. Thereforc. there is a  > 0 such that it follows from u. v e M
and lIu - vII <  that IIAu - A[111 < I.
Then there is an integer n that is independent of U E .W such that one
can partition the segment from 0 to u by subdi\'ision points 140 = O.
UI, ..., u" - u with Ilu. - u.- 1 11 <  for all i; therefore,
"
1/ A II - A (0) II < L d Au; - Au; -I: < n
I-I
for all u E .W.
(III) Show that if the operator A is demicontinuous. then A is locally
bounded.
Othcf\\'ise. there is a u E X and a sequence Un  U with IIAu"tl --+  as
n -t XJ. This is a contradiction to the boundedness of (Au") that follows
from Au,, Au as n  00.
27.4. Seminlonotolle operators are pseudomonotone. Lct X be a real separable rcflex-

610 27. Pscudomonotonc ()pcrators and Quasi-Linear Elliptic Differential Equations
ive a-space. and let the operator 8: X x X ..... X. be given. We set
A'l = B(u. u)
for all u e X.
The operator A: X .... X. is called scmimonotonc iff the follo\\'ing hold.
(i) For all u, t' E X,
(B(u,u) - B(u,v), u - v)  O.
(ii) t'or each u € X, the operator
V'-' 8(u. t)
is hemicontinuous and bounded from X to X. and. for each VEX. the
operator
u...... B(u, v)
is hemicontinuous and bounded from X to X..
(iii) Ifu.u in X as n . and
(8(u., u.) - B(u", u), u. - u) ... 0
as n -t> 00.
then 8(u., v) B(u, v) in X. as n  00 for all VEX.
(i\') Let t: e X. If u"  u in X as n -+ oc,. and
B(u.. v)""'" ". in X.
then (B(u", v), u,,) -t (w, u) as n -+ OCt
(\,) A: X  X. is bounded.
Show that A: X .... X. is pseudomonotone.
Hint: Use similar arguments as in the proof of Proposition 27.6, Cf, Lions
(1969, M), Section 2.5.
as n..... co,
27.5. Exislence theorem for semimonotone operators. Let A: X -t X. be semi-
monotone and coercive (ef. Problem 27.4), Then, for each b e X*, the equation
Au = b.
U EX,
has a solution.
This is an immediate consequence of Problem 21.4 and Theorem 27,8.
27.6. A general cxistence t"eore,,, for quasi-linear elliptic differential equations of
order 2m, We want to generalize Proposition 26.12. As in (26.21). we consider
the boundary \'alue problem
L (- I) [)« As(x. Du(x» = I(x) on G,
S'"
(E)
D'u = 0 on oG
for all fJ: IfJl S m - I.
where G is a bounded region in R'\'. N, m  I. and Du == (Dcru)j"" We set
Du = (du. D",u), where
du = (D'u"slll-I and D.u = (D'U""M'
Let I < p < 00, p-I + q-I -= I. We assume:
(A I) The differential operator of order 2m in (E) satisfies the Caratheodory
condition (H I). the growth condition (H2). and the coerciveness condi-
tion (H4) from Proposition 26.12.

Problcms
611
(A2) The highest order terms are stri,""y monotone with respect to the highest
order derivatives. i.e..
L (A.(x, d, Dill) - A(x. d. D;.»(LY - D) > 0
-'"
for all D", -:;. D;'.. aU d.. and almost all x e G.
(A3) The highest order terms are coercive with respect to the highest order
derivatives. i.e..
I .  Aca(x.d, D",)D A
1m sup  " I = +00
ID",\"'oo cn tJ1-",ID",1 + ID",I -
for almost all x e G and all bounded sets n.
Show that, for each f e L.(G). equation (E) has a generalized solution
u e W,,'"( G) in the sense of Definition 26.11.
Hint: Use the main theorem on scmimonotone operators (Problcms 27.4
and 27.S) and use similar arguments as in the proof of Proposition 26.12. Cf.
Lions (1969, M). Section 2.6.
This theorem dates back to Visik (1961) and Leray and Lions (I96S Further
general results can be found in Browder (1970, S). Compare also Chapter 4
\\'here the relation between (E) and \'ariational problems is studied.
27.7. Quasi-linear elliptic dYJerential equations in unbounded regions. In this connec-
tion, study the work by Hess (1978) and Pascali and Sburlan (1978. M), pp.
299-310. Such problems are characterized by a lack of compactness. For
example, the embedding Wl(G) s; L 2 (G) is not compact ifG ::a (;l'\'. This causes
the typical difficulties for elliptic differential equations in unbounded domains.
27.8. Application of the main theorem on locally coercive operators to periodic solu-
tions of general first-order partial dYJerential equations.
27.8a. Torus and periodic;t)'. Let x = (I'" ., 'N),)' == ('1....., 'l.\,) and p = (PI"'" p,)t
where 0 < Pi < 00 for aU i. Furthermore. let
C = {x ERN: 0 < i  Pi for aU i}
be an N-dimensional cuboid. A function f: R'''' -+ lEI is called p-periodic iff
f(x + p) = f(x) for all x ERN.
If we identify the corresponding points on opposite sides of C, then we obtain
an N-dimensional torus T'\' (d. Fig. 27.2 for N = 2). Each function
f: T". --+ R
is equivalent to a p-periodic function
f: R. tJ ... R.
By definition. the space Ct(T"') consists of all p-periodic C"-functions f: R'" ....
R with the norm
IIfll = max If(x)l.
JtGC
The Sobolev spaces W,i(T"') are defined similarly. For brevity, we set H =
W;( T"').

612 21. Pseudomonotone Operators and Quasi-Linear Elliptic Differential Equations
c
p'!
I
-1- - - - - --
1
PI
I;igurc 27.2
270gb.. Exislellce theorem. We seek a p-periodic solution u: R".  Ii of the gen-
eral first-order partial differential equation
a(x. u(.)t DIl(x)) = f(x) on R N ,
(31)
where D. = oJc; and D = (D... 0" Do")o We make the following assumptions:
(H 1) The function x...... a(x.ll. D) is p-pcriodic on (RN for all (rt. D) € [RN  a and
a e C'.'(T' x R"'+').
s > [N,12] + 30
(H2) a(x.O) = O. There is a positive constant c such that. for all.. e C. }' E IR''',
dcf ca(., 0) I  (; 2 a(x. 0)
A (x) -  - .,  "  C
(1U _ j=-I oxjcD j
and
,2 ,a2a(x.O) 2
A(x)lyl + s l..  aD ttitt.. > clyl ·
JoA -I O.'(J A
(H3) The function f: R N -. R is p-pcriodic and belongs to liS.
Sho\\' that (3 t) is l()Call}' unique/}' solvable. To be precise. thcre arc positive
numbers Rand r so that. for each 1 e liS with 11111,  R, there exists a solution
u e Ha of(31) \\'ith &Ills < r. Moreovcr, the solution u depends on f Lipschitz-
continuousl)' in lis-a-norm.
Hint: Use Theorem 27.8 with Y  X = X" s: Y. and
y = H'I,
X = H',
y of = liS - a .
Let L = (- d)I,'2. Moreo"cr. we set (/Ig) = Jclgdx and
<"'. v)r = (L S - 1 "'IL... 1 v) + ,i2("'lv
('v. t')x = (LS"'IL"v) + i.2("tlv
where i.. is an appropriate real number. 'inally. let
Il.fll .. (f,f)x.
The: space X together with the scalar product (', ')x becomes an H-spacc.
Note that the smooth functions in H J can be represented by Fourier series.

References to the Literature
613
This way. it is easy to provc many properties ofthc spaces H S via Fourier scri
e.g... embedding theorems (cr. Triebel (1972. M), Chapter 6).
We now write the original equation (31) in the form
Au = /. U E X.
Show that thc operators A: X -. Y. and A: Y -+ X arc y,.eakly sequentially
continuous. Moreo\'er, show that. (or sufficiently large i.. we obtain the key
estimate:
c
(Av. v)r  211vll - dllvll.
for all ve Y with Hvllx < I. Set K = {ve X: IJvlJ X S r}. The point is that. for
sufficientl)' small r, relation (32) in1plies the local coerciveness of A. i.e..
(32)
C
(At!, v)r > 2,2 - d,3 ;2:  > O.
foralll'E YncK.
Cf. Kato (1984). A variant of this theorem was first proved by Moser (1966)
via the technique of the hard implicit (unction theorem.
27.8c. GelJeralizal;oll. The preceding result can be generalized to first-order partial
ditTerentia] equations of the form (31) on finite-dimensional, compact,
proper Riemannian manifolds. It is also possible to show that the solu-
tion u of (31) depends continuousl)' on f in the H'.norm. This can be
found in Gunther (1988a) (cr. also Chapter 85).
References to the Literature
Classic,ucd papers: Visik (1963). Lcray and Lions (t96S Brezis (1968).
Pscudomonotone operators and their generalizations: Browder and Hess (1972
Pascali and Sburlan (1978. M), Kluge (1979. M).
Applications to quasi-linear elliptic diffcrential equations: Morrey (1966. tv!). Lions
(1969. M). Browder (1970. S. H). (1986. P). Skrypnik (1973. M (1986, M), Dubinskii
(1976. S. B). Berger (1977. M). Ivanov (1982. S).
Weighted Sobolev spaces and quasi-linear elliptic differential equations: Kufner and
Sandig (1987. M).
Sobolev spaces of infinite order and partial diffcrential equations: Dubinskii (t 984.
M).
Regularity of solutions of nonlinear elliptic equations: Ladyienskaja and Uralce\'a
(1964. M), Morrey (1966, M). Skrypnik (1973. M), (1976. S). (1986, M Giaquinta
(1981, M NeCas (1983" M). Koshelev (1985. L), (1986. M). Giaquinta and Hildebrandt
(1989. M).
Singularities of the solutions of quasi-linear elliptic differential equations: Sernn
(1964), (1965)" Ni and Serrin (1985), Kichenassam)' ( 1987).
Linear elliptic equations in nonsmooth domains: Kondratjev (1967). Kondratjc\' and
Oleinik (1983, S), Grisvard (1985. M). Ku(ner and Sandig (1987, M), Maslcnnikov8
( 1988).
Quasi-linear elliptic equations in nonsmooth domains and asymptotic expansions
near conical points: Miersemann (1982), (1988). (1988a) (applications to the capillary
surface problem).

614 27. P5eudomonotone Operators and Quasi-Linear Elliptic Differential F..quations
Full)' nonlinear equations and the Monge-AmpCre equation: Gilbarg and Trudingcr
(1983. M) (recommended as an introduction). Aubin (1982, M), Evans (1982) (1983),
Lions (1983a, S), (1985), CafTarelli. Nirenberg. and Spruck (1984/88 Lieberman and
Trudinger (1986).
Continuity properties of operators: Vainbcrg (1956, M (1972, M
Main theorem on locall)' coercive operators: Hess (1973), Kato (1984).
Difference method and finite element method for quasi-linear elliptic differential
equations: d. the References to the Literature for Chapter 35.

CHAPTER 28
Monotone Operators and Hammerstein
Integral Equations
Around 1900, Fredholm y,'as the first to study systematically linear integral
equations, by solving linear systems of equations and by passing to the limit. In
this paper. we investigate nonlinear integral equations by solving nonlinear
systems of equations via a variational method and by passing to the limit.
Adolf Hammerstein (1930)
The first application of the concept of monotone operator equations to
Hammcrstcin integral equations was made implicitly by Golomb (1935) and
explicitly by Vainberg (1956).... Our method consists of splitting the linear
kernel operator K \'ia a Hilbert space H and reducing the original equation
II + KFII = O.
II E X*,
with the splitting K = S.CS, to the equivalent equation
C-1w + SFS.w = O. 'E H.
with n' == (S.)-IU, which is then solved by using the results of Brov,'der (1963)
and Mint)' (1963) for monotone operator equations.
Felix E. Bro\\'der and Chaitan Gupta (1969)
In this chapter we investigate the abstract Hammerstein equation
u + KFII = 0,
U E X*,
(1)
with the nonlinear operator F: X. -+ X and the linear operator K: X ...
X., with the aid of the theory of monotone operators and the fixed-point
index. The applications relate to Hammerstein integral equations and bound-
ary value problems for semilinear elliptic partial differential equations.
Whereas, in Chapter 7, we made use of B-spaces of smooth functions and
monotone increasing operators in ordered B-spaces, we now work in L,(G)-
spaces and apply the theory of monotone operators.
615

616
28. Monotone Operators and Hammerstejn Integral Equations
A H ammerste;n ilJtegral equation has the form
u(x) + fG k(x,Y)f(y,u(y»dy = O. (2)
Equation (I) follows from this in the case where we define the so-called kernel
operator K by
(Kw)(x) = fG k(x. y)w(Y) dy.
and \\'hcre F is the NenJycki; operator for f, i.e.,
(Fu)t.) = f(}'. u()t».
I n this connection. the kernel k(.,.) can be regular. weakly singular, or
singular. On application of Lq( G)-spaces, the function U'-' f()', u) must not
increase more rapidly than certain polynomials. If one wants to allow stronger
growth, then one must work with either smooth kernels and B-spaces of
smooth functions (cf. Chapter 7), or one must employ Orlicz spaces for
non smooth kernels (cr. Chapter 53). Nonhomogeneous Hammerstein integral
equations
(2*)
v(x) + fG k(x.y)g(y.v(y»dy = b(x)
can be reduced to the homogeneous equation (2) if one sets
u(x) = v(.) - b(.) and f(y, u()'» = g(), U()1) + b(y».
Analogously, one can reduce the nonhomogeneous operator equation v +
KGv = b to the homogeneous equation (1). Hence it is sufficicnt to consider
homogeneous problems.
Boundary value problems for sen,ilinear elliptic eqrlal;ons also lead to
equations of type (I). For example. we consider the boundary value problem
-u(x)= -f(.,u(.» onG,
II = 0 on aG.
If we define the operator K by u = Kg, where u is a solution of the corre-
sponding linear boundary value problem
(3)
- u(x) = g(.) on G,
u = 0 on oG,
and if F is the Nemyckii operator for f, then there results from (3) the operator
equation
(3*)
u = -KFu,
which coincides with our original equation (I). Here K is called the solution
operator for (3*).
If the boundary oG and the function 9 arc sufficiently smooth, then one can

28. fonotone Operators and Hammcrstein Integral F.quations
617
represent K by means of the classical Green function k(., .) in the form (2*)
abovc. Then the Hammerstein integral equation (2) results from (3).
The approach used in this chapter, concerning the abstract solution opera-
tor K that also comprises generalized solutions of(3*), has the advantage that
it can be applied to problems with nOlJSmoolh boundary oG and nonsI'lOotl,
right member f. In this connection, we do not need any sophisticated investi-
gations concerning the properties of the Green function in the nonsmooth
casc.
J."'or the original operator equation (1) we investigate the following special
cases:
(a) K is monotone and angle-bounded, F is monotone and hemicontinuous
(Theorem 28.A).
(b) K is monotone and compact F is demicontiouous and bounded (Theorem
28. B).
(c) K is monotone, F is pscudomonotone, bounded, and coercive (Theorem
32.8).
(d) K is symmetric (variational method in Chapter 41).
Roughly speaking. the following holds:
The requirements on the JVenJyckii operator F are ,,,eaker, to ti,e extent that
tlU! clssumptiolJs on tile kerlJel operator K are stronger, and con.Jersel}.
In the concrete cases (2) and (3) above. the monotonicity of F means that the
real function
u  /(>1, u)
is monotone increasing for each fixed }: E G. The monotonicity of K corre-
sponds to a certain definiteness property of the kernel k(., .). Moreover, we
consider for equation (I) the convergence of the Galerkin method. In Section
7.13, we have already investigated the convergence of the iteration method for
equation (1).
The basic idea of all proofs of existence for (I) consists in that, in contrast
to (1), one studies the equivalent equations (4), (S and (6) below. We sketch
briefly the arguments used in this connection.
First equit'tllence principle. If we denote by K- 1 the, in general, multivalued
inverse operator to K, then equation (1) is equivalent to -I:" e K- 1 r4, there-
fore also equivalent to
o E K- l u + Fu.
(4)
We make use of this modified equation in (b) and (c). In case (b), the operator
K F is compact. Therefore, we can use the fixed-point index. In fact, let U be
an open ball around the origin. Using (4), we give conditions in Theorem 28.B
guaranteeing that
-tKFu :I: u
for all (14, t) e au x [0,1].

618
28. Monotone Operators and Hammc:rstc:in Integral Equations
Then on the basis of the homotopy H(u.. t) = -IK FII, we obtain for the
fixed-point index:
i( - K f., U) = i(H ( · ,0), U) = I.
Therefore, the operator - KF has a fixed point on U i.e. equation (I) is
sol va ble.
In case (c), the operator K- 1 is maximal monotone. The solvability of
equation (4), and thus of (1), then results from the main theorem on maximal
monotone operators (Theorem 32.A).
Second equivalence pril,ciple. We consider the case (a). If, first of all, the linear
operator K is monotone and s)mmelric on the real separable H-space X, then
K is continuous and there exists a symmetric continuous sqrlare root
S = K 1/2
on X, i.e., K = S2 and S = S.'. If K- 1 exists as a single-valued operator, then
S-1 also exists. Consequently, equation (1) with X = X., is equivalent to
,,, + SFS,,' = 0, we X, (5)
where "7 = S-I U . Let A = I + SFS. If F is hemicontinuous and monotone,
then (SFS"'I v) = (F5"'1 Sv) and, for all ''-I, z eX,
(A,,,. - Azi '" - z) = (M' - zl'''' - z) + (FS,,,' - FSzIS,,, - Sz)  (", - zl ", - z).
Consequently, A is hemicontinuous and strongly monotone on X. The main
theorem on monotone operators (Theorem 26.A) yields a solution of the
equation A,,,. = 0, '" eX, i.e., (5) has a solution '''I. Thus, U = Sw is a solution
of the original equation (I).
More generally, if K: X -+ X. is an angle-bounded operator on the real
B-space X, then we make use of the decomposition
K = S.CS
that is more general in contrast to K = S2 with appropriate operators 5 and
C (cf. the factorization theorem in Section 28.1). Now. equation (I) is equiva-
lent to
C-1w + SFS.w = 0, ", e H, (6)
with '" = (S*)-I U . Here H is an appropriate H-space. Similarly, as in (S one
can apply to (6) the main theorem on monotone operators. The modified
equation (6) also results in the case where K- t docs not exist as a single-valued
operator.
Angle-bounded operators generalize symmetric operators. If K is sym-
metric. then C = 1. The significance of angle-bounded operators consists in
that the solution operators of certain strongly elliptic differential equations
are angle-bounded. Therefore, boundary value problems for scmilinear equa-
tions of type (3), with a strongly elliptic linear differential operator Lu instead
of -u, can be reduced to the original operator equation (I) with angle-
bounded K.
In Chapter 41 (rcsp. Chapter 44) of Part III we deal with the equation

28.1. A factorization Theorem for Angle-Bounded Operators
619
u + KFu = 0 (resp. the eigenvalue problem 14 + )'KFu = 0) for symmetric K
with variational methods.
28.1. A Factorization Theorem for
Angle- Bounded Operators
In order to prepare for Theorem 28.A we construct for the linear operator K
a decomposition of the form
K = S.CS
(7)
\\'ith the following commutative diagram:
K
x
s1 c
H t
bijecliYc
)
X.
S.jIDJccIIVC
H =H*
(8)
Let I denote the identity operator on the H-space II. We make the following
assumptions:
(H I) The operator K: X ..... X. is linear and monotone on the real B-space X.
(H2) The operator K is angle-bounded, i.e., there is a number a  0 such that
I(Ku, v) - (Kv, U>12  4a 2 (KI4, u)(Kv, v) for all u, VEX. (9)
Proposition 28.1 (Browder and Gupta (1969»). Suppose that (HI) and (H2)
hold. Then:
(a) Decomposition. There exist a real H-space H with scalar prodl4cl ( .1. ) and
operators S, C such that (7) holds and tile diagranJ (8) is commr,tatit'e. To be
precise, tile follo\ving hold:
s: X ..... H is linear and continuous \vith IISII 2 < II K II.
Tile rtlnge S(X) is dense ;n H. (10)
C: H ..... H ;s linear, cOIJtinuous, and bijectiL'e. (11)
C - I ;s ske\'-adjoint, i.e., (C - 1)*' = -(C - 1) and
IIC - III  a. (12)
C and C- 1 are strongl}' monotone. To be precise, for all
II E H.
(C- 1 hi h) > (I + a 2 )-l(hlh), (Chlh) = (hlh). (13)
(SuISv) = 2-1«Ku v) + (Kv, u») for all u, veX. (14)
«(C - I)SuISv) = 2- 1 «Ku,v) - (Kv,u») for all u. VEX. (15)
(hISu) = (S*h, U)x for all u e X, h E H. (16)

620
28. Monotone Operators and Hammcrstcin Integrdl Equations
(b) If K :# 0, then
(Ku,u) > (I + a 2 )-1 iIKII-IIJKIlII.
for all u e X.
(c) If K: X  X. ;s strongly contiluloUS, then S: X  H ;s strongl}' continuous.
If K: X --. X* ;s eonlpact on the reflexive B-space X, tllell S: X -. H ;.
compact.
(d) If K is S}'mlnetr;c, thell C = I.
The proof will be given in Problem 28.1. The simple idea of proof consists
in constructing the H-space H with the aid of the bilinear form
[u, v]. = 2- 1 (Ku, t') + (Kv, u» for all u, l e X.
The idea for the construction of the operators Sand C is contained in (14)
and (15).
28.2. Abstract Hammerstein Equations with
Angle-Bounded Kernel Operators
We study the operator equation
u + KFrl = 0,
u EX.,
(17)
\vith the operators F: X. -. X and K: X -. X.. To construct the approximate
solution u,. we use the Galerkin method
(rl" + KFu", w;)x = 0,
; = I. . . . , n,
(18)
with
..
u,. = L cJK.''').
j=1
Letting C = (c1,...,c,,) and gi(C) = (u" + KFu","'i)X' the Galerkin equation
(18) is equivalent to the nonlinear real system
9i(C) = 0,
cERa,
i = I, . . . , II.
For an approximate determination ora solution C \\'e use the iteration method
e(t"'1) = eft) - tg(cC') k = 0, 1, .... (19)
with c(O) = O. Here I is a sufficiently small positive parameter. We make the
following assumptions:
(H I) The operator K: X ..... X. is linear, monotone, and angle-bounded on
the real separable B-spacc X.
(H2) The operator F: X. -+ X is monotone and hemicontinuous. In this
connection, X is identified with a subset of X**.
(H3) Let {WI' \\'2'. ..} be a basis in X and set X" = span {\\'I' . . ., "',,}.

28.2. Abstract Hammerstein Equations
621
Theorem 28.A (Amann (1969a). Suppose that (HI) through (H3) hold. Theil:
(a) Existence and uniqueness. The abstract H ammerslein eqllation (17) has
e.'tCacll}' one solution.
(b) Galerkin method. If dim X = 00, tllell the Galerkin equation (18) lias
exact I}' olle solutioll u. E XII for each n e N. As '1 -. :;x), the seqllence (u II )
converges in the lIorm topolog}' of X* to the solut;oll u of equal;oll (17).
(c) Iteration method. Suppose that tile real filnctions 91'. . .,9" are c:ontilluous/}'
dOerelJtiable Oil IIi" or at least locally Lipschitz continllolls a,ad
det(Kw j , Wj)x) :F 0, (20)
",here i, j = 1,.... n. Tlaen, for sufficientl}' snUll1 and fixed paranleler t > 0,
lile sequence (eC A ) in (19) cont'erges as k -. 00 to the .olution of tile Galerkin
eqllalioll (18).
Note that, according to Theorem 26.8, the value of t can be given explicitly.
Remark 28.2 (Interpretation of X c X.*). In order for the monotonicity of
F: X. -. X to be meaningfully defined, one must think of F as an operator of
the form
F: X* -. X...
We achieve that by X c X... As usual. this inclusion is to be understood as
follows. To each element x e X, one can assign a linear continuous functional
x on X. by means of
x(x.) = x*(x)
for all x* eX.,
i.e., .'tC e X.-. The mapping cp: X -. X.. with q>(x) = x is injective and norm
preserving. We identify x with x. In this sense, X 5 X** and
(Xtx.)x. = (x.,x)x for all x e X, .,* EX.. (21)
In the following proof of Theorem 28.A, we use Proposition 28.1 (splitting
of K) and Theorem 26.A (main theorem on monotone operators).
The reader should always have in mind the splitting
K = S*CS
and the basic diagram (8). This diagram contains tbe operators S: X -. H,
C: H -+ H., S.: H* -+ X., and K: X -. X.. Hence the corresponding dual
operators have the form C*: H.. ... H*,
S..: X.. -. H..
and
K.: X-. -+ X.
where K. = S.C.S...
We identify the H-space H with its dual space H., i.e., \VC set
H* = H. H.. = H.
Then the dual operator C.: H*. -. H* coincides with the adjoint operator

622
28. Monotone Operators and Hammerstein Integral Equations
C.': H -+ H, i.e., we set C. = C.' in Proposition 28.1. Let (.1.) denote the
scalar product on H.
From (16) and (21) we immediately obtain the first important formula
(Svlh) = (v, S*h>x. for all veX, h e H. (22)
By H = H.. and X s; X..t
(S..vlh) = (v,S.h>x. for all veX, II e H.
From (22) it follows that
(S..vlh) = (SvI/J) for all veX, h e Ht
that is,
S..v = Sv
for all veX.
Since K. = S.C.S.., we obtain the seco,ul important formula
K.v = S.C.Sv for all veX. (23)
PROOF OF THEOREM 28.A(a).
(I) Equivalent equation. By Proposition 28.1, K = S.CS. Consequently, the
original equation (17), i.e., U + K Fu = 0, u e X., is equivalent to the
equation
C- 1 ", + SFS.' = Ot '" E H,
with w = (S.)-I U . We set
A,v = C- 1 ", + SFS.,v.
(II) We show that the operator A: H -. H is hemicontinuous. For all h,
\\' e H, it follows from (22) that
(A\\'lh) = (C-1,vlh) + (FS.w, S.h>x..
(24)
Let 'v = u + tv with U t v e H. Then the function t.......(Awl/a) is continuous
on [0, I], since by assumption the operator F is hemicontinuous. Hence
A is hcmicontinuous.
(111) We show that the operator A: H -. H is strongly monotone. Let w, z e H.
By (22),
(Aw - Azlw - z) = (C- 1 (w - z)l,v - z)
+ (FS.w - FS.z, S.w - S.z>x..
The monotonicity of F and (13) imply
(A", - Azl'" - z)  (C-1(w - z)I'" - z)
> (1 + a 2 )-II1'" - zll,
i.c., A is strongly monotone.
(IV) The operator A: H .... H is coercive, since it is strongly monotone.

28.2. Abstract Hammcnlcin Equations
623
Theorem 26.A in Section 26.2 yields that the operator A: H -+ H is
bijective. In particular, there exists exactly one \\,. e H with A". = 0, i.e.,
equation (24) has exactly one solution. Consequently, the original equa-
tion (17) has exactly one solution. 0
PROOF OF THEOREM 28.A(b).
(I) We construct a basis in H. Let
HII = S*-1 K*(X.).
From XII S X II + 1 it follows that H"  H"+I £; H. We shall show that
(ulh) = 0 for all h e U HII implies u = O. (25)
II
Consequently, the set U" H,. is dense in H. If one chooses suitable basis
clements in H", then there arises a basis for the H-space H.
In order to prove (25), assume that (ulh) = 0 for all h e U. HII' Let
v e U" XII' Then
(uIS*-1 K*v) = O.
By (23),
(CuISv) = (1IIC.Sv) = (uIS*-1 K*v) = O.
Since, by assumption, the set U" XII is dense in X and the operator
S: X -+ H is continuous, we obtain
(CuISv) = 0
for all veX.
Moreover, by Proposition 28.1, the range S(X) is dense in H, therefore,
Cu = 0, i.e., u = O.
(II) Galerkin equation for the operator A. We consider equation (24), i.e.,
AM' = O. M' E H. Then the corresponding Galerkin equation is given by
(A"'"I'J) = 0 for all 'I e HII' (26)
We seek "'II e HII' According to Theorem 26.A, equation (26) has exactly
one solution "'" e H,. and
\V" -+ w in H
as n -+ 00,
\\'hcre A \\,. = O. We set
u = S.w
" II
and u = S*,,'. Then we obtain u + K Fu = 0, u e X*, and the continuity
of the operator S* implies
U II  u in X.
as n -+ oc.
(III) Galerkin equation of the original problem. In order to finish the conver-
gence proof for the Galerkin method. we show that U II is a solution of
the original Galerkin equation (18), i.e.,
(U,. + KFu ll , v)x = 0 for all veX.. (26*)

624
28. Monotone Operators and Hammcrstein Integral Equations
Here we seek u" e K*(X,,). More precisely, we show the equivalence of
(26) and (26*).
In fact, by (22), we have
(A\\'"JIJ) = (C-1\\'nIIJ) + (FS*\v", S*h>.t. for all hell".
By (23),
K*v = S*C.Sv
Let veX" and consider
for all v eX..
h = S.-1 K.v,
i.e., h = C.Sv. From (22) it follows that
(C-1"'"IIJ) = (C-1S.-1u"lh) = (C-1S*-IU"IC*Sv) = (S*-l u "ISt')
= (v,u,,>x. = (u",v)x
and
<f.S"\,. S*h>x. = (Fu", S.C.Sv>x.
= (SFu.IC*Sv) = (CSFu.ISv)
= (v, S*CSFu,,)x. = (S*CSFu", v)x = (KFII", v>.t.
In this connection, note that X" c: X S; X.* and use (21). This implies
(A"'"lh) = <U" + KFu", v)x.
From II" = S.-l K*(X,,) and u" = S",,'. with "'" e Hit it follows that
U II e K*(X,,).
Hence (26) is equivalent to (26*).
o
PROOF OF THEOREM 28.A(c). We set
.
v =  c.w.
L J l'
j=l
II = K.v
,
and
gj(C) = (II + KFu, "'i>x.
We want to apply Proposition 26.8 to the Galerkin equation
gee) = 0,
To this end, we need the key condition
e e R".
"
L (gJ(e) - 9J( e »(c J - c J )  i'lic - c [12
J=I
for all c, c e A" and fixed i' > O. To this end, we set
aCe, c ) = (II, v >x.

28.3. Abstract Hammerstein Equations with Compact Kernel Operators
625
By (21), (KU.l)x = (v,K,,>x. for all u, VEX. From this it follows that
a(c, c ) = (K*v,v)x = (v, K v )x.
"
= (K v, v)x = L (K\v i , "j>.y Cj cJ'
i.j-1
and
a(c, c) = (Kv,v)x  0,
since K is monotone.
Let a(c, c) = O. Then (Kv, v) = O. By Problem 28.2b, this implies Kv = 0,
.
I.C.,
II
(Kv, "J> = L (K,,'., "J)XCi = 0
i-1
for j = I, ..., n. Hence we obtain e = 0 since det«K"'''''i)x)  o. Con-
sequently, the bilinear functional a(., .) is positive definite, i.e., there is a )' > 0
such that
ate, e)  )' II ell 2
for all e e: W.
Now the key condition above follows from the monotonicity of F. In fact, we
obtain that
"
L (gj(c) - gj(c))(C j - Cj) = (u - II, V - v)x + (KFu - Kf. ", v - t' )x
j1
= (u - ii, v - v>x + (Fu - F", u - ii>.f.
 (u - u, v - v )x
= a(c - c,c - c)
/lIc- c tl2.
Now Proposition 26.8 yields the convergence of the iteration method C C1 + 1 ) =
('(k) - tg(C(A) for the equation gee) = o. 0
28.3. Abstract Hammerstein Equations with
Compact Kernel Operators
We consider again the abstract Hammerstein equation
u+KFu=O, ueX., (27)
with the operators F: X. ..... X and K: X -+ X*. In contrast to Theorem 28.A,
\ve now require the compactness of K. However. we can weaken the assump-
tions on F, in particular. we do 1I0t require F to be monotonc. In this
connection, we formulate the following two conditions:

626
28. Monotone Operators and Hammerstein Integral Equations
(C 1) K is monotone, i.e. t
(K "', '''')x  0
for aU w EX,
and there exists a number R > 0 such that
(u, Fu)x > O. for all u e X. with lIunx. = R.
(C2) There exisl a number }' > 0 such that
(K M', \\. > X  I' II K ,,, II i. for a II W EX.
Moreover t there exists a function cp: III. -. R and a number R > 0 such
that cp(R)/R 2 < )' and
(u,Fu)x  -cp(lIull) for all u e X*.
By Proposition 28.1 (b), the requirement on K in (C2) is fulfilled in the case
where K is angle-bounded.
Theorem 28.8 (Hess (1971) and Amann (1972». The abstract Hammerste;n
equation (27) has a solution i" the case where the following three assulnptions
are fil(fil'ed:
(i) Tile operator K: X -. X. is linear and compact on the B-space X.
(ii) Tile operator F: X* -+ X is demicontilJUor,s alul boululed.
(iii) Either (C I) or (C2) is valid.
PROOF. We will use the properties (AI), (A2), and (A4) of the fixed-point index
in Section 12.3. We set
u = {u e X*: lIuli x . < R}
for fixed R > O. Below we shall show that the operator KF: X. -. X. is
cOlnpact and that
-tKFu #; u
for all (u, t) e au x [0, 1].
(28)
Then the assertion follows from standard arguments. In fact, letting H(u, t) =
- tK Fu, the homotopy invariance (A4) of the fixed-point index yields
i(H(.,O), U) = i(H(., 1), U).
Since H(u,O) = 0 and 0 E U, we obtain from (At) that
i(H ( · , 0), U) = I,
and H(u, 1) = - KFu implies
i(-Kf.,U) = I.
Now the existence principle (A2) yields the existence of a fixed point u E U
of the operator -Kl-e. Therefore, the equation KFu + u = 0, u e U, has a
solution.

28.4. Application to Ilammerstein Integral Equations
627
(I) We show that the operator KF: X. -+ X* is continuous. From
u.. .... u in X.
as '1.... 00,
it follows that
FUnFu in X
as n.... 00,
since F is demicontinuous. The operator K: X -+ X* is linear and com-
pact and hence strongly continuous. This implies
KFu" -. KFu in X. as n -. 00.
(II) We show that KF: X. -. X. is compact. Let (u ll ) be a bounded sequence
in X*; then (Full) is bounded in X, since F is bounded. Because of the
compactness of K there exists a subsequence (un') such that (KFu lI ,)
converges in X..
(III) We prove (28). If (28) does not hold, then there exists a point u E au and
ate [0, 1] with - tKFu = u. Because U :t- 0, we have t > 0 and there
exists awe K -1 (u) \vith
", + tf"u = O.
(29)
In the case (CI), from (K,,', w)x  0 and Kw = u, it follows that
(u, w)x  0 and hence
<u w)x + t(u Fu)x > o.
This contradicts (29).
In the case (C2 we obtain that
(u, \v)x + r(u,J-"u)x > IIIull 2 - cp(JluD)
= R2(i' - lp(R)/R 2 ) > o.
This also contradicts (29).
o
28.4. Application to Hammerstein Integral Equations
We consider the Hammerstein integral equation
u(x) + fG k(x. Y)f(Y. ub')) dy = O.
u e Lp(G).
(30)
Our goal is to obtain an overview of a series of different conditions on the
kernel k(x, y) and the nonlinearity fey, u) that guarantee the existence of
solutions for (30). We set
X = Lq(G
I < q < 00.
We then have
X. = L,(G),
p-I + q-I = 1.

628
28. Monotone Operators and Hammerstein Integral Equations
In order to be able to apply our abstract results, we write (30) in the form
u+KFu=O. aleX.. (31)
In this connection, the linear operator K: X -+ X* is generated by the kernel
k( ., · ), i.e.,
(Kv)(x) = fe; k(x.y)v(y)d}'.
The operator F is the Nemyckii operator for f, i.e.,
(Fu)()') = f(), u(}'».
We formulate the following four basic assumptions:
(H 1) G is a bounded region in A. v with N  I,and I < p, q < 00, p-l + qui = 1.
(H2) L;nearit) and monotonicit)1 of K. The kernel k: G x G .... IJi is so con-
stituted that the operator K: X .-. X. is linear, i.e., K tt e X. for all
t' E X. This implies
(Kv. w>x = fe; [fe; k(X.Y)V(}')d Y ] w(x)dx
Moreover. let K be monotone, i.e.,
(Kt\ v>x > 0 for all VEX.
(H3) Gro\\,th condition for f. The function f: G x IR -. R satisfies the Cara-
thcodory condition (e.g., f is continuous) and there is a nonnegative
function a E Lq(G) and a number b  0 such that the growth condition
Lf(}', u)1 S at.v) + b lul,,-I
holds for all (.v, II) E G x R.
(H4) The functions {"'., "'2'. .. } form a basis in X = L 4 (G).
In addition, we will make use of one of the following two conditions:
(HS) Coerciveness of f. There is a number d > 0 such that
for all v, \\' e X.
f()p, u)u  dl ul P for all ()', u) e G x (R.
(HS*) As}mptotic positivity of f. There is a number R > 0 such that
fey, U)II  0
holds for all (y. II) E G x R with lul  R.
We say that f is IIJOIIOIOne (resp. strictly monotone) with respect to u iff the
function
u H fey, u)
is monotone increasing (resp. strictly monotone increasing) on R for all } E G.
Recall that K: X .... X* is stricti)' InonOlOlJe iff
(Kv, t')x > 0 for all nonzero t' E X.

28.4. Appli"'tioD (0 Hammerstein Integral Equations
629
Moreover. the linear monotone operator K is angle-bounded iff there exists a
number a  0 such that
I(Kv, }\-')x - (K}\-', v)xr < 4a 2 (Kv, t:)x(K,,', }v)x
for all v, '" E X.
In particular, this condition is satisfied in the case where K is symmetric, i.e.,
(Kt', }\-')x = (K}v, v)x
for all t', )\. EX.
(32)
STANDARD EXAtPLE 28.3 (Operator K). Suppose that (H 1) holds and suppose
that the kernel
k: G x G --. IR
is continuous. Let X = Lq(G) and X. = Lp(G). Then:
(i) The operator K: X -+ X. is linear and compact.
(ii) The dual operator K*: X .... X. is given by
(K*v)(x) = fG k(y,x)v(y) d)' for all veX.
(iii) Let k(x, )') = k(), x) for all x, Y E G. Then K: X -+ X. is symmetric, If K
is monotone, then K is also angle-bounded.
Let p = 2, i.e,. X = X. = L 2 (G). Then the compact symmetric operator
K: X .... X has a complete orthonormal system of eigenvectors on the H-spacc
X. If all the eigenvalues of K are nonnegative (resp. positive), then K is
monotone (resp. strictly monotone).
In Corollaries 28.5 and 28.6 below we will generalize this well-known result
to regular kernels k e Lp(G x G) and to weakly singular kernels,
In order to compute approximate solutions u" of the Hammerstein integral
equation (30)" we apply the Galerkin method. The Galerkin equation (18)
corresponding to the operator equation (31) reads as follows:
fG [un(X) + fG k(X,Y)!(}I,u,,(Y»dY]Wi(X)dX:: 0 (33)
for ; = 1, . . . , n with
"
u,,(x) =  cJ(K.wJ)(x),
This is a nonlinear real system of n equations for the n unknown real numbers
Cl'....C".
Proposition 28.4 (Solution of the Hammerstein Equation (30) for a Monotone
Kernel Operator K). Under the assumpt;olls (HI), (H2). (H3), and (H4) the
follo\ving assertiolJS are valid:
(a) Convergence of the Galerkin method. Suppose that K is angle-bounded
(e.g., s}'tmmetric) alld f is n,olJotoIJe ",;tIJ respect to u.

630
28. Monotone Operators and Hammerstein Integral Equations
Then equation (30) Iws exactly one solution u and, for each n. the Galerkin
equation (33) has exactly one solution II" \vhere the seqllence (u,,) converges
;11 L,(G) to u as II ..... 00.
(b) Existencc. Equation (30) IttlS a soll4tion in the case ,,'here one 01 the lollo\ving
tlwee conditions is t'alid:
(i) f is 1)JO,Joto'le ,,'ith respect to II and satrU!s the coerciveness condition
(HS). r p = q = 2. then (H5) drops out.
(ii) K is conapact and f satisfies (HS).
(iii) K is compact and angle-bounded and f salisfies the asymptotic posi-
t ivit}: condit ion (H S*).
(c) Existence and uniqueness. Equation (30) has exactl}' one solutio" in the case
\vhere one of the follo\vi'19 two conditions is (,l;d:
(i) 1 ;s stricti), ,nonOlone \Vilh respect to u and .alisfles the coerciveness
conditio'l (HS). If p = q = 2, tllen (H5) drops 0111.
(ii) K is stricti}' monotone. and f ;s monotone \v;th respect 10 u and salisfies
(H 5).
Stronger results for symmetric kernel operators K can be found in Section
41.6. There we will use a variational approach.
PROOF. We usc, in an essential way, the structure theorems on the Nemyckii
operator in Section 26.3. 8y the growth condition (H2 the Nemyckii operator
F: X* -+ X is continuous and bounded and
(u.Fu>x = fo It(y)f(y.u(y»dy
If f is monotone (resp. strictly monotone) with respect to u, then F: X. ..... X
is monotone (resp. strictly monotone).
The coerciveness condition (HS i.e.,
for aU u e X*.
uf()', II) > d I IllP
for all (y,l4) e G x R
implies
(u, Fu)x  d fG lul'dy = dUull.
i.e.!, F is coercive.
The asymptotic positivity condition (HS*) implies
<u, Fu)x > -c for all u eX.,
where c is a positive constant. This follows from Proposition 26.7.
Now, the assertions of Proposition 28.4 follow from our results on abstract
Hammerstein equations.
Ad(a). Cf. Theorem 28.A in Section 28.2.
Ad(b), (i). cr. Theorem 32.8 in Section 32.5 and Theorem 32.0 in Section
32.23.
for all II EX.,

28.4. Application to Hammcrstcin Integral Equations 631
Ad(b), (ii). cr. Theorem 28.8 in Section 28.3 with condition (Cl).
Ad(b), (iii). Cf. Theorem 28.8 with condition (C2).
Ad(c). Cf. Theorem 32.8 and Theorem 32.0. 0
In the following three corollaries to Proposition 28.4 we summarize several
well-known results concerning linear integral operators of the form
(K v)(x) = fG k(x, y)v(y) d..\',
In this connection, we generalize Standard Example 28.3 and \ve obtain
results which are useful for applying Proposition 28.4 to broad classes of
Hammerstein integral equations.
(H) Let G be a bounded region in R-\' with N > I and let 1 < p, q < OCt with
p-I + q-J = I. We set X = Lf(G). Then X. = Lp(G).
Recall that L,(G x G) consists of all measurable functions k: G x G -+ IR
with
f. 'k(., ))IP dx dy < oc.
GxG
Moreover, by definition, k e Lcr.(G x G) iff k: G x G -t (R is measurable and
bounded (e.g., continuous and bounded).
CoroUary 28.5 (Regular Kcrnels and Compact K). Suppose tllat (H) holds and
t IUI' tlte kernel k is regllltlr, i.e., k e L,,( G x G).
Tllen tile operator K: X ..... X. is linear and compact a,ul the dual operator
K*: X -+ X. is given b}1 tile relatiolJ
(K*v)(x) = fG k(}', x)v(y) dy.
Jor almost all x E G and all v e X*. In particular, if
k(x. y) = k()', x)
for al,nost all x. y e G,
then the operator K: X  X. is s}'nnnetr;c.
PROOF. For example, see Kantorovic and Akilov (1964, M), Section 2.
o
Corollary 28.6 (Weakly Singular Kernels and Compact K). Suppose lllat (H)
holds and that ,he kernel k is weakl}' singular, i.e.,
k ( ) = na(x, ))
x, y I 1 2 '
. - )'
o < :x < N.
Jor all x, ) e G \vith x #: }'. Here, m e L«;.(G x G) and N denotes tI,e dimension
of G. Let X = Lq(G), 1 < q < roe

632
28. t-fonotone Operators and Hammcrstein Integra) Equations
Then tile operator K: X ... X is linear and conapaCI.
If 2  q < oc., then 1 < p S 2 a,ul tlJerejore, the en,beddi'lIJ X c X. is C01l-
ti'UIOIIS. Hence tile linear operator K: X ..... X. is conapact. Tile dual operator
K.: X -.. X. has the same properties as in Corollar}' 28.5.
PROOF. For example, see Triebel (1972, M), p. 122.
o
We now consider the limit case !X = N, i.e., we consider the singlilar kerllel
c(x s)
k(:<.y) = I I N'
x-y
\vherc s = (. - }')/Ix - YI. The function c(.. .) is called the characteristic of
the singular kernel. We have S E S, where
U(.\;,t) = {)' e [RN: I}' - xl < £}, S = {x E [RN: Ixl = I}.
For almost all x E G. we define the integral operator K by the limit
(Kt')(x) = Iim f. k(x, y)v()) dy,
t-O G-(l(.t:)
i.e., the integral is to be understood in the sense of the Cauchy principal value.
Corollar). 28.7 (Singular Kernels and Continuous K). Suppose that (H) holds
,,,-illt N > 2 a"d that tlae kernel k ;s s;ngillar, i.e., C ;s nlea.urable a'Jd
Is c(x. s) dO(s) = 0 for all x E G.
sup f Ic(x, s)IP dO(s) < 00.
EG S
Let X = Lq( G). I < q < 00.
Theil the lillear operator K: X  X is continuous.
If 2  q < 00, the'3 tile embedd;,'9 X c X* ;s conti1luous. Hence tile linear
operator K: X .... X* ;S COllt;nrIOUS.
This is the famous theorem of Calderon and Zygmund (1956) which plays
a fundamental role in the modem theory of linear elliptic partial differential
operators. Corollary 28.7 remains valid for G = AN.
28.5. Application to Semilinear Elliptic
Differential Equations
We consider the boundary value problem
-II(X) = -f(x,u() on G,
II = 0 on oG,
(34)

28.5. Application to Scmihncar Elliptic Differential Equations
633
Of, more generally,
Lu - Jll' = -/(x, u) on G,
DTa, = 0 on aG for all }': li'l  m - 1,
\vith the fixed real number Jl and the 2nJth order linear differential operator
Lu = L (-I)pIDor(a_D"u).
121.1-1 s '"
We make the following assumptions:
(H I) G is a bounded region in IR N , N > 1.
(H2) All the functions a«,: G -+ IR are measurable and bounded. and the
ellipticity condition (E) below is satisfied.
(H3) The function f: G x iii -. R satisfies the Caratheodory condition (e.g.. f
is continuous), and there exists a nonnegative function a E L 2 (G) and a
positive number b such that the following growth condition holds:
I.f(x, u)1  a(.) + biu1 for all (x. u) e G x IR.
(35)
Definition 28.8. Let X = W 2 -(G). Then the generalized problem for (35) reads
as follows: We seek a function u e X such llaat
a(u. v) = - fG f(x. u(x))v(x)dx
for all v e X,
(36)
\\'here
a(ll, v) = f. r (atJ,UvDlJ u - IlUV) dx.
G 1«1.1111 s; III
As usual, the generalized problem (36) results from (35) if one multiplies (35)
by v e Co(G) and then integrates by parts. In particular. the special case (34)
corresponds 10 m = 1 and
f. ,..
a(u, v) = r D;14D,vdx,
G ;=1
where x = (1"'" N) and D f = O/af' As a further important assumption we
formulate the following elliplicit}' condition:
(E) There exists a number c > 0 such that
a(u,u)  cllulli
(37)
for all u e X.
In the special case (37 condition (E) is valid because of the Poincare-
Friedrichs inequalit}'.lf the linear differential operator L is strongly elliptic,
then it follows from the Garding inequality in Section 22.15 that
a(l4,u) > cUull - (C + p)ilull
for all" e X and fixed c > 0, C e R. Consequently, condition (E) is valid for
all Jl with Il  - C.

634
28. Monotone Operators and Hammcrstein Integral Equations
If, in addition, L is symmetric, then condition (E) is valid for all p with
Ii < Jlmin' where Pmin is the smallest eigenvalue of the eigenvalue problem (35)
with f = o.
Proposition 28.9. Under the assumptions (HI)-(H3), the follo,ving hold:
(a) Existence and uniqueness. Suppose that the !unctio'J u t-+ f(x, u) is monotone
i,u:reasi'Jf1 on [R ,for all x e G. Then the generalized problenl for (34) (resp.
(35» has exactl)' one solution.
(b) Existence. Suppose tllat there exists a nun,ber R > 0 sllch tlJat
f(x,u)1I  0
for all (x, u) E G x R '\lith I III  R.
(38)
The" tlJe ge'Jeralized problel11 for (34) (resp. (35» has a solution.
PROOF. Ad(a). Let Y = L 2 (G). Then Y. = L 2 (G). By Section 26.3, the Ncmyckii
operator F: Y. ..... Y corresponding to f is continuous, bounded, and monotone.
We consider the linear boundary value problem
Lu - pu = g on G,
D"u = 0 on oG for all )': Jyl S m - I,
i.e., we set - f(.'t, u(.'t» = g(x). By (E) and Theorem 22.C the corresponding
generalized problem (36) has exactly one solution u. We set
II = Kg.
By Corollary 22.20, the solution operator K: Y ... Y. is linear, monotone,
compac and angle-bounded.
Consequently, the generalized problem for (35) is equivalent to the operator
equation
u = -KFu,
u e Y*.
(39)
Now Theorem 28.A yields the assertion.
Ad(b). By Proposition 26.7, it follows from (38) that
(u, Fu)y  - c for all u e Y.,
where c is a positive constant. Theorem 28.B with condition (C2) yields the
assertion. 0
PROBLEMS
28.1. Proof of Proposition 28.1.
Solution:
(I) Construction or the H-space H with the scalar product ( .1. ). As a linear
monotone operator, K is continuous. We construct the two bilinear
functionals
[u. t]! = 2- 1 «Ku. v) :f: (Kv. u».
for all u. f] € X.

Problems
635
Note that [ " · ] + is symmetric and [., . ] _ is skew-symmetric. It follows
from the monotonicity of K that
[u.u]... = (Ku,u)  0
for all u eX.
According to the Schwarz inequality for positive symmetric bilinear
functionals, we obtain
I [u, v] ..1 2 S [u, u] + [v, IJ] +
for all u.. I e X
(40)
(ef. Problem 21.16). The operator K is angle-bounded. This means
I [u. V]_12  a 2 [u. u] t [v. v]. for all u. veX. (41)
We now consider the zero set
z - {u E X: [u,u]. = O}.
By (40) and (41 [u, v]t = 0 for u e Z or v E Z. This implies
[u + "', v + z] = [II.. v]! for all u. veX, "', z e Z. (42)
The key to our proof is the equivalence relation
u""v
iff
u - V E Z.
Let X /Z denote the set of equivalence classes arising this way. We equip
the factor space X /Z with the scalar product
dff (
(UI V) = u, v].,
(43)
where u e tl and v e  By (42 this definition is independent of the
choice of the representatives u e U and v e  Moreover, ( .1' ) is indeed
a scalar product on X /Z, for it follows from (UI U) = 0 that [u.. u]. = 0,
i.e., u e Z, t hercfore U = O.
According to the completion principle, we can extend XjZ to an
H-space II such that
x /Z  H
and X/Z is dense in H (see Problem 18.4).
(II) Construction of the canonical operator S: X  H. We define
5u = U,
ue U,
i.e., the operator 5 assigns to each u E X the corresponding equivalence
class U. Therefore,
(SuISv) -- (u,v]. ;;; 2- 1 «Ku,v) + (Kv,u»).
The operator S is linear and continuous, for
 S u II  = (Sui Su) = < K u, u) S I! K u 1111 u IJ SilK 1111 u 11 2 ,
and hence 11511 2  IlK II.
(III) Construction of the operator B: H -+ H. For all U, Ve XjZ.. we define
b(U, Y) - [u, v]_,
where u e V,
V E It:
By (42), this definition is independent of the choice of the representatives

636
28. Monotone Operators and Ilammel'$tein Integral Equations
u e U and v € J.-: From (41) it follows that
Ib( [l, V)I < a II [/11 H II V · H
forall U. Ve XjZ.
(44)
Since X/Z is dense in H. wc can extend b(',') to II x H such that (44)
holds for all U.. V E H. Consequently, there exists a linear continuous
operator 8: H -.. H with
b(U, V) ;: (BUI V)
forall U.VeH.
(45)
By (44). "811  Q. Because b( U. V) = - b( I', U), wc have
B.. = -B,
i.e.. B is skew-symmetric. It follows from (45) that
(BSuIBv) = b(Su, St') == [u, v]_
for all u, veX.
(46)
(IV) Properties of the operator (.. = 1 + B.
(IV-I) C is strongly monotone, for (BUIu) = b(U, U) = 0 and thus
(CUIU) = «I + B)UIU) = . VII;, for all [/ E H. (47)
(IV-2) C is bijectivc. In fact. because IIB S a.
.C.V 11 1 = «I + B) VI(I + B) V)
= II Vd 2 + IIBVn 2
« 1 + a 2 )IIV'2 forall VeH. (48)
From !lev II  II VII for all V E H it follows that C is injective and C(H)
is closed. We show that C(H) = II. If we had C(/I) 1/1. H, then there would
exist V :;= 0 with (CUI V) = 0 for all U e H. This means that (eVI V) = 0,
therefore V :3 0 by (47). This contradicts V :I: O. Thus C(H) = H.
(IV-3) C- 1 is strongly monotone on II. For, by (47) and (48
(VIC-IV) ' (C(C-IU)IC-IU) = lIC- l l/11 2
 (1 + a 2 )-1IiUll 2 for all U el,.
(V) Propcnics of the dual operator S*: H* -. X*.
(V -I) By the definition of the dual operator and H- :: H. we obtain that
(hISu) = (S.h.u)x
for all u E X, h E H.
(49)
(V -2) S* is injective. For, b)' (49 it follows from S.h = 0 that (hISu) =
(S*h.u) = 0 for all u e X. Since SeX) = XjZ is dense in II, we have
h = o.
(V -3) The norm of a linear continuous operator is always equal to the norm
of the corresponding dual operator. Hence IISII = IIS*II.
(VI) The decomposition K = S.(1 + B)S follows from
(Ku,v) = [u,v] + [u,v] = (SuISv) + (BSuISv)
= «I + B)SuISv)
= (S*(1 + B)Su, v) for all u, t' E X.

References to the Literature
637
Equation (48) together ,,'ith liS. II = : 511 yields
IIKuJ2 = IIS.CSuIl 2 S IISII 2 11CSull 2
< IIKII(I + a 2 )IISu1l 2 = ilK 1(1 + a 2 )(Ku,u).
This is Proposition 28.1 (b).
Let K be strongly continuous. Then, from u" -At u as n -+ 00, it follows
that Ku" -+ Ku and hence
IISu" - Sul1 2 = <Ku. - Ku,u,. - u).-. 0
as n.... J:J"
i.e." SUn'" SUo Therefore" S is strongly continuous.
Let K: X .... X. be compact on the renexive B-spacc X. Then S: X .....
H is also compact, since a linear operator on a reflexive B-space is
compact iff it is strongly continuous.
If K is symmetric, then [u, v] _ = 0 for all u. L' eX. i.e.. B = O.
The proof of Proposition 28.1 is complete.
28.2. Linear mOlJotone angle-bounded operators.
28.2a. let K: X  X. be a linear and monotone operator on the B-space X. Show the
equivalence of the follo\\'ing t\\'O assertions:
(i) K is angle-bounded, i.e., for all u, v E X and fixed a > 0"
I(Ku, v) - <Kv, u)1 2 s: 4a 2 (Ku, u)(Kv" v).
(ii) For all u v.: e X,
(Ku - Kz.: - v) S 4- 1 (1 + a 2 )(Ku - Kv,u - v).
Hint: cr. Pascali and Sburlan (1978, M), p. 191.
28.2b. Let K: X -. X. be a linear monotone angle-bounded operator on the B-space
x. Show that (K\\., ",) = 0 implies K,,' = o.
Solution: We set z = t' + I)'. t > 0 and u = \\- + V. By (ii) above,
(Ku - Kz.z - v) S O.
Letting I -. O. we obtain < K "'. }') < 0 for all y eX. i.e.. K ". = O.
References to the Literature
Classical work: Hammerstein (1930).
General expositions on Hammerstein integral equations: Krasnoselskii (1956, M
(1966" M), Zabreiko and Krasnoselskii (1975. M)" Vainberg (1956, M) (197 M),
Pogorzelski (1966, M), Pascali and Sburlan (1978. M). Guseino\' (1980. M).
Angle-bounded monotone operators and Hammerstein integral equations: Amann
(1969), (1969a), (1972), Browder and Gupta (1969), Pascali and Sburlan (1978. M).
Monotone operators and Hammerstein integral equations: Browder (1971" S" B),
Hess (1971), Vainberg(1972, M Brezisand Browder (1975). (1918). Brezisand Haraux
(1976), Pascali and Sburlan (1978, M).
Monotone operators, singular integral equations, and nonlinear boundary value
problems in complex function theory: v. \Volfersdorf (1982), (1983)" (1987), Wegert
(1987) (topological methods).

638
28. Monotone Operators and Hammerstein Integral Equations
Galcrkin method: Amann (1969a), Vainberg (1972, M).
Numerical methods: Amann (1972a). Delves and Walsh (1974, M)(numerous physi.
cal applications Fenyo and Stolle (1982. M Vol. 4, Hackbusch (1985, M) (multigrid
methods), Reinhardt (1985, M).
Subroutine package QUADPACK for automatic integration: Piessens (1983).
Monotone operators and nonlinear integral equations of Levin - Nohel t)lpe: Barbu
(1976, M).
Handbook on linear and nonlinear integral equations: Zabreiko and Krasnoselskii
(1975).
(Cr. also the References to the Literature for Chapter I (integral equations), Chapter
7 (scmilincar equations), and Chapter 53 (integral equations in Orlicz spaces).

CHAPTER 29
Noncoercive Equations,
Nonlinear Fredholm Alternatives,
Locally Monotone Operators,
Stability, and Bifurcation
It came as a complete surprise. when. in a shon note published in 1900.
Fredholm sho\\'ed that the general theory of all integral equations considered
prior to him was. in fact, extremely simple.
Ivar Fredholm (1866-1927) was a student of Mittag-Leffler in Stockholm in
1888-1890; he published only a few papers during his lifetime.. mostl)' concerned
\\'ith partial differential equations. After a visit to Paris, where he had been in
contact with all the French analysts. and had become familiar with the recent
papers of Poincarc.. he communicated, in August 1899, his first results on integral
equations to his former teacher: they were published in 1900 and completed 1\\'0
years latcr in a paper published in Acta Mathematica.
Jean Dieudonne (1981)
The purpose of this note is to introduce a nonlinear version of Fredholm
operators, and to prove that in this context Sard.s theorem (1942) holds if zero
measure is replaced by first category.
Steve Smale (1965)
These notes formed the basis of a course entitled "Nonlinear Problems" given
at the Department of Mathematical Analysis, Charles University, Prague.. dur-
ing the years 1976 and 1977.
The problems of solvability of noncoerc;ve nonlinear equations and of non-
linear Fredholm a)ternati\'es have been very popular recently. Therefore, it was
the author's desire to give a survey of results concerning these problems which
are known at present, as well as to show the methods which ha\'e been used to
obtain them and some of their consequences for concrete problems.
Svatopluk Fuak (1971)
Svatopluk FuCik died prematurely on May 18, 1979. He was 34 years old and
knew since 1973 that his time was severely limited. 1973 is also the year when
Futik wrote the first of twenty-one papers devoted to nonlinear noncoercive
problems. . . . This domain of analysis owes so much to his remarkable ingenuit),
and formidable energy.
Jean Mawhin (1981)
639

640
29. Noncoerci\Oe Equalions and Nonlinear Fredholm Altcrnati\'cs
We consider the operator equation
Au = b, u e: X.
The main theorems on monotone and pseudomonotone operators in
Chapters 26 and 27, respectively, ensure the existence of a solution u for each
h E X* if the operator A: X -. X. is coercive. In this chapter we want to study
the case that A is Ilot coercive. Here we need solvabilit}' conditions for the right
member h. In particular, we will study the asymptotically linear operator
equation
BfI + N u = b, u eX, (I)
i.e., the operator B is linear and continuous. and the nonlinear operator N
satisfies the condition
lim IINull = O.
1..1-':(1 lIuli
Roughly speaking, we obtain the following results:
(i) If the linearized equation Bu = 0 has only the trivial solution u = O. then
equation (1) has a solution for each be X*.
(ii) If BIl = 0 has a nontrivial solution u :;: 0, then (1) has a solution if b satisfies
an appropriate solvability condition.
Statement (i) can be formulated like this: If the linearized equation Bu = b
has at most one solution Il, then the nonlinear equation Bu + Nu = b pos-
sesses a solution u. Roughly speaking, that means:
Uniqueness implies existence.
This principle is of general interest because, as a rule, it is much easier to prove
the uniqueness of solutions than the existence.
Concerning the nonlinear operator N, we consider the following two cases:
(a) R(N) s; R(B) (Theorems 29.A and 29.C);
(b) The operator B + N has so-called weak asymptotes (Theorem 29.D).
The applications of (a) and (b) concern integral equations and boundary value
problems for semilinear elliptic equations, respectively. In connection \\'ith (b).
we consider theorems of the so-called Landesman-Lazer type in Section 29.9.
Here, we obtain the surprising fact that the necessar}' solvability conditions are
also sufficient.
The general idea of proof is the following:
() We replace equation (1) by an equivalent problem, where we do not
assume that the single-valued inverse operator B- 1 exists. In Section 29.1,
we will study several different possibilities for fonnulating such important
equivalent problems.
(fJ) We apply the mapping degree to the equivalent problem corresponding
to (I). In particular, we will use the Borsuk antipodal theorem and the
Leray-Schauder principle from Part I.

Noncoerci\'c Equations and Nonlinear Fredholm Alternati..,es
641
Further important results on the range of sum operators can be found in
Section 32.22.
In Section 29.10, we study in detail the operator equation
(E)
AI4 = b,
u e S,
where A is a proper IJon/i"ear Fredllolm operator and S is a subset of a B-space.
The class of Fredholm operators plays a fundalnental role with respect to
integral equations, differential equations, and the calculus of variations. We
want to show what the abstract operator equation (E) behaves like in the
special case of reasonable real functions A: R ... IR. This is a crucial
observation. In particular, we will show that, in most cases, equation (E) has
at most afinile number of solutions u, and the number of solutions is locally
COI,stan, with respect to the right member h. Roughly speaking, the funda-
mental Sard-Smale theorem tells us that:
Paliiological bellavior 0.( equalioll (E) is rare with respect to tile rigllt menl-
ber b.
In Section 29.11, we introduce the notion of locall}' nlonotone operators.
This class of operators allows important applications to the calculus of varia-
tions and to elasticity. In Sections 29.12 through 29.22, we invcstigate the
following topics:
(i) sufficient conditions for strict local minima Oocally regularly monotone
operators, the abstract GArding inequality, the Hestenes theorem, and
eigenvalue criteria);
(ii) nonlinear Fredholm operators and an abstract stability theory;
(iii) a general bifurcation theorem for operators of variational type;
(iv) local and global multiplicity theorems for nonlinear Fredholm oper-
ators.
\Ve want to emphasize the following general principle:
Loss of stab;l;t) can lead to bifurcation.
In connection with applications to the calculus of variations it is important
in (iii) that the so-called operators of "variational type" gelleralize potential
operators. This way, it is possible to prove a general bifurcation theorem for
Euler-Lagrange equations in spaces of smooth functions. In contrast to the
corresponding theory in Sobolev spaces, we do lIot need any restrictive growth
conditions for the differential equations.
Bifurcation theory studies equations of the form
(B)
G(f4, b) = 0,
ue X
be P,
where the parameter" b lives in the parameter space P. Obviously, equation
(B) generalizes the equation Au = h, which we have considered above. Let

642
29. Noncoercive Equations and Nonlinear Fredholm Altcrnati\'cs
u 1 u
X
"0 r 
I  h "' b
b o
(a) (b)
Figure 29.1
G(1I0' b o ) = 0, and let the operator
G: U(Uo, b o ) c X x P ..... y
be C 1 in a neighborhood of the point (r4 0 , b o ), where X, P, and Yare real
B-spaces. Suppose that the linearization
G.,( r4 o t b o ): X -. Y
is a Fredholm operator of index zero. Then, according to Sections 8.1 and 8.4,
we have the following two different situations:
(a) Regular case. If GII(uo, bo)h = 0 implies h = 0, then G.,(r40, b o > is bijective
and the solution set of (B) in a neighborhood of (uo, b o ) is given by a
CI-curve u = u(b) through the point (uo, b o ). This follows from the implicit
function theorem (Fig. 29. t (a).
(Il) Singular case. If the linearized equation G.(uo, bo)h = 0 has a nontrivial
solution h :/: 0, then the solution set of (B) in a neighborhood of (u o , b o )
may have a complex structure. In particular, it is possible that this solution
set bifurcates at ("0' b o ) (Fig. 29.1 (b».
In terms of elasticity, we have:
u = displacement of the elastic body;
b == outer forces.
Roughly speaking, the regular case () means that the outer Corces cause
uniquely determined displacements of the body, whereas bifurcation in (fJ)
corresponds, for example, to the buckling of rods, beams, plates, and shells.
In Sections 29.13 and 29.19, we consider:
(a) applications of bifurcation and stability theory to the buckling of beams;
and
(b) applications of the general theory for minimum problems to the calculus
of variations.
In particular, we study the following in (b):
the Legendre-- Hadamard condition and strongly eUiptic systems, strongly
stable solutions oCthe Euler-Lagrange equation, and sufficient conditions for
local strict minima;

29.1. Pscudoresolvcn Equivalent Coincidence Problems
643
the Jacobi equation and sufficicnt eigcnvaluc criteria for local strict minima;
the continuation method and an approximation method for solving the
Euler - Lagrange equations in the domain of stability;
loss of stability and a gencral bifurcation theorem for Euler-Lagrange
equations;
stability of the bifurcation branches.
Applications of these results to nonlinear elasticity will be considered in
Chapter 61.
Our abstract results represent the final functional analytic formulation of
classical results due to Euler, Lagrange, Legendre, Jacobi, and Fredholm,
mentioned in Section 18.1. Roughly speaking, we obtain the following:
Euler-Lagrange equation => necessary conditions for local InilJima of
fllnctionals;
Legendre condition => locally reglilarly monotone operators alJd
sufficiellt COlulitions for mininJQ of funclionals;
Jacobi equation => sufficielJt eigenvalue criteria for local "JilJinJa
of functiolJQls;
Fredlaolm.s theory of  nonlinear Fredholm operators.
integral equatiolJs
29.1. Pseudoresolvent, Equivalent Coincidence
Problems, and the Coincidence Degree
We consider the semilinear operator equation
Bu = Mu, u E D(B)  D(M), (2)
where B: D(B)  X ... Y is a linear operator and JW: D(J\{) S;; X -+ Y is a non-
linear operator. This equation is called a coincidence equation because one
wants to find a point u for which thc images under Band M coincidc. If the
inverse operator B- 1 : Y -. X exists, then equation (2) is equivalent to u =
B- 1 Mu. However, in this section, we consider operators B which are not
necessarily invertible and we want to obtain a number of different problems
which are equivalent to (2). Those equivalent problems are very useful in order
to prove existence theorems for (2). Our goal is to show the connections
between apparently completely different approaches in the literature. To this
end, we shall use the pseudoresolvent as a simple tool. Before considering the
general case, we begin with an important special case.
We make the following assumptions:
(H 1) X and Yare B-spaces over (K = R, C and M: X -+ Y is a givcn operator.
(H2) The linear continuous operator B: X ..... Y is Fredholm of index zero with

644
29. Noncoercivc Equations and Nonlinear Fredholm Alternatives
dim N(B) > 0, i.e., the range R(B) is closed and
dim N(B) = codim R(B) < oc.
We studied Fredholm operators in Section 8.4. Recall that dim N(B*)
= dim lv(B). The dual operator B* R.aps y* into X*.
(H3) Let {III'...' u lI } and {fir,..., u:} be a basis in N(B) and N(B.), respec-
tively. Note that Iii e X and Ul. e Y. for all i.
(H4) By A,(SI), we choose elements Vi e Yand Vi. E X. such that
<u,.,v J > = <v,.,"}) = 'J" i,j = 1, ... tn. (3)
and we set
II
Qu = L <v,., u)u"
jel
II
Pb = b - L <u,.,b)v i .
j=1
Rccall that the equation
Bu = b,
UE X,
(4)
has a solution iff
<u.,b) =0 forall u*eN(B* (5)
i.e., Ph = b. Consequently, the operators Q: X  N(B) and P: Y  R(B) are
projection operators onto the null space l'J(B) and the range R(B) of B,
respectively.
No\\' we are ready to give our ke}' definition:
"
Su = Bu + L <Vr,U)Vi.
'=1
(6)
We shall show below that S: X -+ Y is bijective. Hence the inverse operator
S-I: Y -+ X exists. This operator is called the pselldoresoltnl of B. Moreover.
we shall show below that the following problems are mutually equivalent:
(A) Original equation
Bu = M u,
U EX.
(7)
(8) Fi.'-:ed-poinl problem of E. SchnJidt (1908)
"
II = S-I Mu + L <vf,u)u;.
1=1
U E x.
(8)
(C) Branching equatiolas of E. Schmidt (1908)
"
u = S-I Mu + L CiU,
i-I
(9a)
c, = (v,., u),
We seck u E X and CI'...' C. E ()(.
i = I,... , n.
(9b)

29.1. Pscudorcsolvcn Equi\'alent Coincidence Problems
64S
(D) Bra'ic/,ing equations of Ljapunov (1906)
w = S-I PJW(I' + \\'), (lOa)
o = (1 - P)M(v + w). (lOb)
We seek v e N(B), '" e N(B)l. and set u = v + w. Here, N(B)l ==
(1 - Q)(X).
(E) Alternative problem of Cesar; (1964)
.
14 = S-1 J\l14 + L CiU.,
.=1
Ci = c. - t(u! t M,4),
j = It..., n.
In this connection, let t e IK be a fixed nonzero number. We seek u e X
and C I , . . . , ell elK.
Obviously. this problem is equivalent to the following problem:
(11 )
CI=cl-t\Ur,M(S-lMU+ j CjUj)),
We seek u e X and c., ... , C n e IK.
(F) Alternative problem of M a ",hi'l (1972)
u = S-I ( MU - r. (u,.. MU)f)j ) + f. (ur, Mu) Uj + f. (vt. u)u r . (12)
1-1 ;81 i=1
"
u = S-1 Mu + L C,Uj'
'-I
Proposition 29.1. Assume (H 1) through (H4). Then:
(a) The operator S: X --. Y is a linear homeomorphism.
(b) If b e R(B), then BS- 1 b = b.
(c) Problems (A) through (F) are mutuall} equivalent.
Before giving the proof, we want to discuss this result. In (C) one first solves
equation (9a). Then the solution u = u(c 1'. . . , c n ) has to be substituted into
(9b). This yields the branching equations of E. Schmidt
C, = < v,. , u(c 1 ,. . . , C n », i = 1, . . . , n.
Similarly, in (D) one first solves equation (lOa). Then substitution of the
corresponding solution \\' = ",(v) into (lOb) yields the branching equation of
Ljapunov
(1 - P)M(v + ",(v» = 0,
v e N(B).
Sometimes it is better to solve (lOb) first and to substitute the solution
v = v(,,') into (lOa).
In order to solve the equations (B)-(F) above and the more general equa-
tions (B*)-(F*) bclo\v, one can use fixed-point theorems, mapping degree, the

646
29. Nonooercive Equations and Nonlinear Fredholm Alternatives
theory of monotone operators, variational methods, etc. Many papers in the
literature are based on this approach, in order to prove a steadily increasing
number of existence theorems for nonlinear ordinary and partial differential
equations. Our approach should help the reader to understand the substance
of the turbulently accumulating literature.
Remark 29.2 (Coincidence Degree). If one wants to apply the Leray-Schauder
mapping degree from Part I to the original problem (A) above, then one can
proceed as follo\vs. We consider the equivalent fixed-point problem (B) of
E. Schmidt and we set
"
Cu = S-I Mu + L (vr, U)U i .
i-I
Then the equation
Bu = Mu, U e G,
is equivalent to the equation
Cu = U, II e G.
Suppose that Bu #: Mu on aGe This implies Cu #= u on aGe Moreover, suppose
that C: X -. X is compact (e.g., M is compact). Then the Leray-Schauder
degree deg(l - C, G) is well defined and we can define the so-called coincidence
degree by means of
dcg(B, M; G) = deg(l - C, G).
(13)
One can show that the following hold:
(i) If we fix an orientation in both N(B) and N(B.), then deg(B, JW; G) does
not depend on the choice of the positively oriented basis {u I' . . . , u II } and
{ur,..., u:} in "r(B) and N(B-), respectively.
(ii) If we change the orientation in either N(B) or N(B. then deg(B. M; G)
changes its sign.
Using(B*) and (F*) below, it is possible to generalize the coincidence degree
in a simple \\'a)' to more general situations. This will be studied in detail in
Problems 29.1 through 29.4.
The coincidence degree was introduced by Mawhin (1972) in order to prove
systematically existence theorems for differential equations (e.g., the existence
of periodic solutions).
PROOF OF PROPOSITION 29.1. Ad(a). The operator B: X -+ Y is a Fredholm
operator of index zero, and S: X -+ Y is a compact perturbation of B. Hence
S is a Fredholm operator of index zero. Thus, in order to prove the bijcctivity
of S, it is sufficient to show that Su = 0 implies u = o.
Let Su = o. Then
"
Bu = - L (vr, U)Vi.
i-I

29.1. Pscudorcsolvent. Equivalent Coincidence Prob1cms
647
From (u:,Bu) = (B*u:,u) = 0 for all k it follows from (3) that (v:,u) = 0
for all k and hence Bu = O. This implies u = L;c;u;, Finally, we obtain from
(3) that
C i = (v:,u) = 0
for all k,
i.e., II = O.
Thus, the operator S: X ..... Y is bijective and S-I: y -.. X is continuous by
the open mapping theorem A I (36). By definition of S, we obtain Sill = t'I' i.e.,
S-l Vi = u. for all i.
Ad(b). Let bE R(B). Then (u:,b) = 0 and (U:,BII) = 0 for all U E X and
all k.
Let u = S-1 b. Then Su = b and hence
.
Bu = b - L (vr, u)v,.
.=1
Applying u: to this equation, we obtain from (u:.v;) = tS. i that (_':,u) = 0
for all k, i.e., Bu = b. Thus. BS- 1 b = b.
Ad (c).
(I) (A)  (B). By definition of S, equation (A) is equivalent to
..
Su = JWU + L (vr, U)Vi'
6=1
This is equivalent to (B).
(II) (B)(q(E)(F). This is obvious. For example, if u is a solution
of (E), then (ur. Mil) = 0 for all i, since I  O. Hence Mu e R(B). Apply-
ing the operator B to the first equation of (E), we get Bu = Mu, since
Bll; = 0 and BS- 1 Mil = Mil, according to Proposition 29.1 (b).
(III) (A)<:>(D). We set u = v + '" ,,'ith v = Qu and \\. = (I - Q)II. Then (A) is
equivalent to the system
B(v + ",) = PM(v + \\'),
(I - P)M(v + \\.) = 0,
and, in turn, this is equivalent to
S\\,. = PJ\1(v + \to'),
(1 - P)kt(v + ".) = O.
Note that Bv = 0, and that Q\\" = 0 implies (vr, \\.) = 0 for all i. 0
The approach above is convenient for treating concrete problems because
we have explicit constructions at hand. However, in order to get more insight,
we now consider a geometrical approach for a more general situation. The
basic idea is contained in Figure 29.2.
Note that the following considerations contain the situation above as a
special case, if we set
Ju. = v.
1 "
i= l.....n.

648
29. Noncocrci,'c Equations and Nonlinear ".rcdholm Alternatives
iXl
X J r:::oI:)«
Y = R(B).l  R(B)
projections
y
1\
Y = R(B) E9 R(B)
S 5 JQ + B
Figure 29.2
We make the foUowing assumptions:
(H 1 *) X and Y are B-spaces over K = R, C, and the operator M: D(M) 
X -. Y is given.
(H2*) The linear operator B: D(B) s X -. Y is Fredholm of index zero, i.e.,
the range R(B) is closed and
dim N(B) = codim R(B) < 00.
(H3*) Let
Q: X -+ N(B),
p: Y -+ R(B),
be projection operators onto N(B) and R(B), respectivel}'.
Such operators always exist because dim N(B) < 00 and codim R(B) < 00.
\Ve set Ql = I - Q and pi = I - P as well as
N(B) = QJ.(X) R(B)J. = p.l( Y).
This yields the topological direct sums
X = N(B) ED N(B).l, Y = R(B) Et> R(B).l.
Rccall that
Qu = u iff u e N(B)
and Qu = 0 iff u e N(B)!. Hence Q2 = Q. It follows that the operator
B: N(B)! r. D(B) -+ R(B)
is bijective. In fact, Bu = 0 and u e N(B)J. imply u e N(B), i.e., u = O. Let
K: R(B) -+ N(B)l "D(B)
be the corresponding inverse operator, i.e., BKb = b on R(B).
(H4.) Let J: N(B) -+ R(B)l be a bijective linear operator. Such an operator
always exists because (H2*) implies that dim N(B) = dim R(B).l < 00.
Now we are ready to give our ke}' definition:
Su = Bu + JQu
for all u e D(B).

29.1. Pseudoresolvenl, Equivalent Coincidence Problems
649
We shall show below that the operator S: D(B) -. Y is bijective and
Su = Bu for all u E N(B)l. n D(B).
Hence
S-lb = Kb
for all b E R(B).
Moreover, we shall show below that the following problems are mutually
equivalent:
(A *) OrigilJal equat;o'J
Bu = M
u e D(B)r. D(M).
(B*) Fixed-point problenJ of E. Schmidt
u = S-I Mu + Qu,
(C*) BranchiPJg eqllatioPJ of E. Schmidt
u = S-I J\l11 + v,
II e D(M).
v = Qu.
We seck II e D(J\f) and v e N(B).
(D.) Branching eqllaliolJ of LjapulJov
S-l PM(v + ".) = \v, pi M(v + \v) = o.
We seek v e N(B), \\-. e N(B)l. and set u = v + w.
Since S-l b = Kb on R(B), this problem is identical with
KPM(v + \v) = "', pl. M(v + ",) = o.
(E*) Alternative problem qfCesari
u = v + S-Ift-tu,
v = v - tJ- 1 pi Mu.
Here let t e K be a fixed nonzero number. We seek II e D(M) and
 e N(B).
Obviously, this problem is equivalent to
u = I' + S-I Mu. v = v - rJ- 1 pl. M(v + S-I Mu).
(F*) AlterPJatil..'e problem of Mawhin
u = S-1 PMu + S-I pl. Mil + Qrl.
As we shall show, this problem is the same as
u == KPMu + J- 1 pi Mu + QII, U E D(J"I).
Proposition 29.3. Suppose that (H I *) through (H4*) hold. Then tlU! operator
S: D(B) -.. Y ;s bijective a,ul
Srl = Bu on N(B)l n D(B), Su = Ju on N(B).
Problem. (A *) through (F*) are mutually equivalent.

650
29. Noncocrci\'c Equations and Nonlinear Fredholm Alternativcs
PROOF.
(I) S is injective. In fact, if Su = 0, then it follows from Srl = Bu + JQII and
Bu e R(B), JQu E R(B)l, that
Bu = 0,
J Qu = O.
This implies U E N(B) and Qu = 0, i.e., II = O.
(II) S is surjective. To show this let ye 1': Then there exists a decom-
position
y = b + c, b e R(B), C E R(B) I .
Let u = Kb + J-Ic. Then. by the definition of S and Figure 29.2,
Su = SKb + SJ- 1 c = b + c = )'.
(III) Note that Q2 = Q. From SQ = JQ it follows that
S-lJQ = Q.
Furthermore. from J- I = QJ- 1 and hence SJ- 1 pl = JQJ- 1 pI = pol.
we obtain
S-1 pl = J-I pl..
(IV) (A *) <=- (8*). Equation (A *) is equivalent to
Su = Mu + JQu,
and, in turn, this is equivalent to
u = S-J Mu + S-I JQu = S-1 J\fu + QII.
(8*) <:> (C*) <:> (E.). This is obvious.
(B.)(F.). This follows from S-Ib = Kb on R(B) and S-I pl =
J-I pl..
(A *) <:> (D*). This follows as in the proof of (A) <=- (D) above. 0
29.2. Fredholm Alternatives for Asymptotically
Linear, Compact Perturbations of
the Identity
We consider the nonlinear operator equation
811 + Nu = b.
together with the linearized problem
Bu = b,
ue X,
(14)
U E X.
(IS)
We set B = 1 + L.

29.2. Fredholm Alternatives for As)'mptotically Linear Operators
651
neorem 29.A (Kacurovskii (1970». Suppose that:
(i) The operators L, N: X -+ X are cOlnpact on rile B-space X.
(ii) L is linear, and L + N is asymptotically lillear, i.e., IINuli/liuli -+ 0 as
li 1411 -+ cc.
Then:
(a) If Bu = 0 implies u = 0, tllen equation (14) has a solution for each be X.
(b) If R(N) c R(B) then equation (14) has a solution u iff b E R(B).
By (i), the operator B: X -+ X is Fredholm of index zero. Thus, R(N) c R(B)
iff
(u*, Nv) = 0 Cor all u. e N(B-}, veX. (16)
Moreover, b e R(B) iff
(u.,b) =0 Cor all u-eN(B-). (17)
PROO... We will use the pseudoresolvent S-l corresponding to B and the
fixed-point index introduced in Chapter 12.
Ad(a). If N(B) = {O}, then R(B) = X. Consequently, (a) is a special case
of (b).
Ad(b).
(I) Suppose that (14) has the solution u. Then b E Nu + R(B), i.e., b e R(B).
(II) Conversely, let b e R(B). We want to show that equation (14) has a
solution.
(11-1) Modified equation. Suppose that u is a solution of the equation
Su + Nu - b = O. (18)
Since R(N)  R(B) and b e R(B), we get Su e R(B). By Proposition
29.1 (b), Su = Bu. Hence BII + Nu - b = 0, i.e., u is a solution of(14).
(11-2) Solution of(18) via homotopy. We may suppose that X :;: to}. Define
H(u, t) by the relation
u - H(u.t) = Su + t(Nu - b),
where 0  r  1, and the operator S is defined by (6) if N(B)  {O}.
In the special case N(B) = {O} let S = B. Since S = B + compact, we
obtain that the map
H: X x [0, 1] ..... X
is compact. By Proposition 29.1, the inverse S-l: X ..... X is linear and
continuous.
Let U = {u e X: lIuli < R}. For sufficiently large R we have the key
relation
H(u, t) :;: u for all (u, r) E au x [0, 1]. (19)
Indeed, this follows from lIuli = liS-I Sull S; liS-III II Su II and hence
Ull - H(u, t)j;  US-Ill-I Dull - "Null - IIbll,

652
29. Noncoerci\'e Equations and Nonlinear Fredholm Altcrnati\"CS
noting that flNuli/liuli -+ 0 as 111411 -+ 00. By the homotopy invariance
(A4) of the fixed-point index in Section 12.3, it follows from (19) that
i(H(.,O), U) = i(H(., I). U).
Since 1''-' H(II, 0) is odd, we obtain i(H(., 0), U) =1= 0, by the antipodal
theorem in Section 16.3. Hence
i (H ( ., 1), U) :F o.
According to the existence principle (A2) in Section 12.3, the equation
14 = H(u, I 14 e U, has a solution, i.e., Su + Nu - b = o. 0
29.3. Application to Nonlinear Systems
of Real Equations
We consider the nonlinear system
"
L bJ) + N( l' · · · , ,,) = b,
j-l
i = 1,..., n.
(20)
For given b = (hI'. . .. bIt) in R", we seek x = (I". .... .) in Di". We make the
following assumptions on the nonlinearities N l :
(H I) Growth condition. The functions N.: (Ji"  [R are continuous for all i.
There are numbers c > 0 and 0   < 1 such that
I N(x)1  clxl. for all x e R" and all ;.
(H2) Orthogonality condition. We have
"
L rNi(X) = 0
i-I
for all . e (R" and all solutions ( ,. . . , :) of the dllallinear system
n
L rb'J = 0,
1=1
j = 1, . . . , n.
(20*)
Proposition 29.4. Lellhe real (n x n)-malrix (b i ) be givelJ.
(a) Assllnze (H 1) and det(bij) #: o. Then, for each b E R", equation (20) has a
Solulion.
(b) AssullJe (H 1) alld (H2). Then, equation (20) has a solution x e R" iff
n
L rbi = 0
'=1
for all solutions (r,..., :) of (20*).

29.5. Application to Differential Equations
653
PROOF. By (HI), IN(x)l/lxl  constlxl«-l -+ 0 as Ixl-+ 00. Now, the assertion
follows from Theorem 29.A with X = (R". 0
29.4. Application to Integral Equations
Let -00 < a < b < 00. We consider the Hammerstein integral equation
u(t) - L& k(t,s)[u(s) + f(s, u(s»] ds = g(t
together with the linearized equation
u(t) - f: k(t, s)u(s) ds = 0,
a < t < b,
(21)
a < t < b.
(22)
Proposition 29.5. Suppose that:
(i) Thefi"lctionsk:[a,b] x [a,b]-+lRandf:[a,b] x R-+Rarecontinuous,
and there clre numbers c > 0 and 0  a < 1 such lllar
I/(s,u)1 clul(l for all (s,u)e[a,b] x R.
(ii) The linearized equation (22) has only the trivial solution u = 0 in C[a, b].
Theil, for each 9 E C[a,b], equalion (21) has a solution II in C[a,b].
PROOF. Set X = C [a, b] and
(Nu)(t) = - f.: k(t, 5)1(5, U(5» ds.
By (i).
IINrl1l = max I(Nu)(t)1 < c(b - a). max Ik(ts)llIuIl2.
ClSISb dr..sb
Hence IIlvull/liuli -.0 as lIuli -+ 00. Now use Theorem 29.A(a).
o
29.5. Application to Differential Equations
Let -00 < a < b < 00. We consider the semilinear boundary value problem
- uN(t) + q(t)u(t) + 1(1, U(I» = h(t),
u(a) = u(b) = 0,
together \\'ith the linearized problem
-11"(1) + q(t)U(I) = 0, a < I < b.
u(a) = u(b) = o.
a < t < b,
(23)
(24)

654
29. Noncoerci\'c Equations and Nonlinear Fredholm Altemati't"CS
Proposition 29.6. Suppose that:
(i) Thefunctionsq: [a,b] -. Rand/: [a,b] x IR -. R are continuous, and there
are numbers c > 0 and 0 S « < 1 such that
If(l, u)1 S clul« for all (t, u) E [a.b] x R.
(ii) The linearized problem (24) has only the trivial solution u = 0 in C 2 [a, b].
Then, for each h e C[a, b], the original problem (23) has a solution u e
C2[ab].
PROOF. Let k(.,.) be the Green function corresponding to the differential
operator Lu = u" with the boundary conditions u(a) = u(b) = O. Then prob-
lem (23) is equivalent to the integral equation
u(t) = 1& k(t, s)(q(s)u(s) + f(s, u(s» - h(s»ds.
Note that k: [a, b] x [a, b] -+ R is continuous. Now, the proof proceeds
analogously to the proof of Proposition 29.5. 0
29.6. The Generalized Antipodal Theorem
In order to generalize Theorem 29.A to noncompact operators, we need a
generalization of the antipodal theorem. To this end, we consider the operator
equation
Bu + Nu = b,
together with the Galerkin equations
(Bu. + NUll' \'i) = (b, 'v,),
ue X,
(25)
u lt EX..,
;= l,...,n,
(26)
where X n = span {WI'. . ., 'v,,}, i.c.,
..
U lt = L c,-W..
i-I
Here we seek the real coefficients Cta. We make the following assumptions:
(HI) The operators B, N: X -+ X. arc bounded and dcmicontinuous on the
real separable reflexive B-space X with dim X = 00. Let {WI' \"2'. . . } be
a basis in X.
(H2) B is odd, i.e., B( - u) = - B(u) for all 14 E X.
(H3) For fixed b E X. and each t E [0, I], the operator A, defined by
A,u = Bu + t(Nu - b)
satisfies condition (S)o on X.
(H4) The decisive homotopy condition. There is an R > 0 such that
At u #; 0 for aU u E X with lIuli = R and all t E [0, 1].

29.6. The Generalized Antipodal Theorem
655
Theorem 29.8 (Generalized Antipodal Theorem). Assume (HI) through (H4).
Tllell:
(a) Existence. Equation (25) has a solution u ,,'ith lIuli < R.
(b) Galerkin method. There is an no such that, for eac/J n > no, the Galerkin
eqllat;ola (26) has a solution u" e X" with lIu,,1I < R. Tile sequelJce (u,,)
pos..e..es a Slibsequence ",/aicIJ converges strongl}' in X to u.
If the original equation (25) has a unique solution u '\lith lIuli < R, then
the entire sequence (u,,) cont't!rges to u.
PROOF. According to Proposition 27.4 it suffices to prove that there is an no
such that, for each n  no, the Galerkin equation (26) has a solution II" with
IIII"N < R. To this end, we will use the classical antipodal theorem in finite-
dimensional spaces.
We set U" = {u eX,.: lIuli < R} and
"
H,,(u, t) = U - L (A,u, Wj > 'Vi.
f1
Our goal is to solve the equation
H"(u,,, I) = U",
u. E V".
(27)
That means < A I U.., 'Vi) = 0 for i = I, . . .. n; therefore, u. is a solution of the
Galcrkin equation (26).
(I) The decisive homotopy property. We will show below that there is an
no such that
H,,(u, t) :I: u
(28)
for all (II, t) e cUlt x [0, I] and all n  no.
(II) Solution of (27). From (28) and the homotopy invariance (A4) of the
fixed-point index in Section 12.3 it follows that
;(H.(., O V.) = i(H.(., I), V,,).
By (H2), the map U'-' H,,(u. 0) is odd. The antipodal theorem in Section
16.3 teUs us that i(H..(. t O'h u.) =F 0 and hence
i(H..(., l u.)  o.
By the existence principle (A2) in Section 12.3. equation (27) has a
solution.
(III) Proof of (28). Let E.: X" ... X be the embedding operator correspond-
ing to X" c X. Then, the dual operator E:: X. -+ X: satisfies
(E:u,v)x n = (u,E"v)x = (u,v)x (29)
for all u E X., v e XII.
(III-I) We first prove the following. For each t E [0, 1], there is a bet) > 0 and
an no such that
II E: A,ull  <S(t)
for all u e au", "no.
(30)

656
29. Nonooercive Equations and Nonlinear Fredholm Alternatlvcs
Otherwise, there is a t and a sequence (u..) with
II E:A,u,..1I -. 0 as n' -+ 00,
and II u".11 = R for all n'. For brevity, we write u" instead of UII. By (29),
I (A,ii.., u,,) I = I (E: A,u,, u,,) I
S IIE:A,u"IIR --.0 as n  00. (308)
Similarly, for all K' e span {\".' "'2.. . . }, we obtain
(A,u", ",)  0 as n  00. (30b)
Since (u,,) is bounded, there is a subsequence, again denotcd by (fl,,).
such that
u u
"
as n -+ 00.
Since Band N are bounded, the sequence (A,",,) is bounded. By (30b).
Aru.O
as n -+ 00
(cf. Proposition 21.26(c) (f»). The operator A, satisfies condition (s)o.
Thus, it follows from (30a) that
U" -+ u
as n -+ oc.
This implies
A,II = o.
In this connection, note that A, is demicontinuous, since Band N have
this property. Furthermore, lIu II = R. This contradicts (H4).
(111-2) We show that  and 1J0 in (III-I) can be chosen independently of t.
Indeed, note that HE..II = I and hence IIE:U = IIE"II = 1. Moreover,
note that Band N are bounded. Thus. for each '0 e [0, 1], there exists
an open neighborhood U(t o ) of '0 such that c5(l) and no(t) can be chosen
as constants on U(lo). Now the assertion follows from the fact that the
compact set [0, 1] can be covered by finitely many open sets U(lo),
U (I I ). . · · .
(111-3) The norm of the functional
,,,....... (A,u, \v)x on X"
is equal to liE: A,ull. Indeed, it follows from (29) that
tiE: A,ull = sup (E: A,II, w)x = sup (A,u, "')x.
"
1"'11 S 1 Iwlx S 1
A ft
By (111-2), there is an no such that
E: A,II #= 0
for all u e au", 1 e [0, 1], n  110. Thu we obtain
(A,II, "'i) #= 0
for some i.
This implies (28).
o

29.8. Weak As)"mptotcs and Fredholm Alternatives
657
29.7. Fredholm Alternatives for Asymptotically
Linear (S)-Operators
We consider the operator equation
Bu + Nil = b,
U EX,
(31)
and make the following assumptions:
(H 1) The operator B: X -. X. is linear and continuous on the real separable
reOexive B-space X.
(H2) The operator N: X -. X* is demicontinuous and bounded. Moreover.
B + lv is asymptotically linear, i.e.. II Nu II/trull -. 0 as II ull -. 00.
(H3) For each b e X. and each t e [Ot 1], the operator II Bu + t(Nu - b)
satisfies condition (S) on X.
Theorem 29.C (Hess (1972». Assume (HI) through (H3). Then:
(a) Suppose, Itat Bu = 0 implies u = O. Then, for each b e X., eqllatioll (31) has
a solutioll u.
(b) Suppose I hat R(N)  R(B). Then, equation (31) has a solution u ijJ b e R(B).
(c) The operator B is Fredllolm of illdex zero.
PROOF. Assertion (c) will be proved in Problem 29.5. Now the proof proceeds
analogously to the proof of Theorem 29.A by replacing the classical antipodal
theorem with the generalized antipodal theorem (Theorem 29.B). In this
connection. note that the operator
II...... Su + t(Nu - b)
(32)
is a strongly continuous perturbation of u Bu + t(Nu - B), according to
(6). By (H3), the latter operator satisfies condition (S). Thus, it follows from
Figure 27.1 that the operator (32) also satisfies condition (S) and hence
condition (s)o. 0
Since B is a Fredholm operator of index zero, we have R(N)  R(B) iff
(u.,Nt') =0 for aU u*eN(B*), t,eX,
and b E R(B) iff
(u.,b) = 0
for all u* e N(B*).
29.8. Weak Asymptotes and Fredholm Alternatives
We again study the operator equation
Bu + Nu = b, u e X. (33)
We want to relax the condition R(N) c R(B which we employed in the

658
29. Noncoercive Equations and Nonlinear Fredholm Alternati\"C5
preceding sections. Roughly speaking. we will use the asymptotic condition
(vi N(v + \\J» = a(v) + o( 11(,'11)
as II v II ..... OC,
for all v E N(B) and all w e X, in order to obtain the necessary and sufficient
solvability condition:
a(v) > (vi b)
for all v E N(B) - {O}.
(34)
More precisely, we make the following assumptions:
(H 1) The operator B: X ..... X is linear, continuous, and self-adjoint on the real
H-space X. The range R(B) is closed, and 0 < dim N(B) < 00.
(H2) The operator N: X -+ X is compact and sup{UNull: u e X} < 00.
(H3) The operator N has weak asymptotes on the null space N(B) of B, i.e.,
for all v E N(B) and \ve X, we have the decomposition
(vIN(v + ",» = a(v) + rev. \v),
I . Ir(v, \\J)I 0
1m sup = ,
IvJ"' wcl: II vII
for all bounded sets K in X, and
(35)
where
a(tv) = la(v)
for all t > 0, v e N(B).
Theorem 29.D (New (1973». Assume (HI) through (H3). LeI be X be give".
Then:
(a) If conditio" (34) is satisfied, then tile original equation (33) has a solution u.
(b) COrlverselYt if (33) has a solution u and if r(v, ",) < 0, i.e.,
a(v) > (vIN(v + \\J» for all v e N(B) - {O}, \\J e X, (36)
then conditio" (34) is satisfied.
Corollary 29.7. The same is true if we replace «> " by "<" in (34) and (36).
EXA1tIPLE 29.8. Suppose that (HI) holds with dim N(B) = I and let e be a unit
vector in N(B). Moreover, set
Nv = h«vle»e
for all v e X,
and suppose that the function h: IR ..... R is continuous and the finite limits
h( + (0) = lim h(s)
s ... :t to
exist (Fig. 29.3). Then. the conditions (H2) and (H3) are satisfied if we set
h( +oo)(vle) if (vie) > 0,
a(v) = h( -oo)(vle) if (vie) < 0,
o if v = O.

29.8. Weak Asymptotes and Fredholm Alternatives
659
h( + (0)
s
h
- - - - - - - - - - - - - - - - - II( - 00)
Figure 29.3
By Theorem 29.D, the equation Bu + Nil = b, "e X, has a solution if (34)
holds, i.e.,
h( -(0) < (ble) < h( +00)
in the case where h( -00) < h( +(0) (resp.
h( +(0) < (ble) < h( -00)
in the case where h( +00) < h( -(0). This sufficient solvability condition is
also necessary if
h( -00) < h(s) < h( +00) for all S E IR
(resp. h( +00) < h(s) < h( -ex) for all S E R).
PROOF OF THEOREM 29.D. \Ve combine the alternative method of Cesari in
Section 29.1 with the Leray-Schauder principle in Chapter 6. The necessary
a priori estimates follow from the existence of weak asymptotes and from the
solvability condition (34).
Ad(a).
(I) The equivalent fixed-point problem. Using the Identification Principle
21.18, we set X = X*. Then, (u*, v) = (u*lv) and B* = B. Let S-I: X -.
X be the pseudorcsolvcnt to B introduced in Section 29.1. Set
g(u) = S-I(b - Nrl).
We now use the alternative method ofCesari. By (11) and Proposition
29.1, the original problem (33) is equivalent to the fixed-point problem
u = i.(v + g(u»,
C, = l[Ci - t(u,IN(v + g(u» - b)],
i = 1, . . ., n,
(37)
with ,{ = 1 and fixed t > O. Here {" 1 t . . . t "It} is an orthonormal basis in
the null space N(B), and
JI
V = L C,U f .
'=1

660
29. Noncoerci,'c Equations and Nonlinear Fredholm Alterna1i,,-es
Equation (37) is equivalent to
u = ).(v + g(u» u E X, V E N(B
v = ). ( V - t r. (1;14; ) ,
;=1
(37*)
\vhere
i = (u.IN(v + g(u» - b).
We write (37*) in the form
(u, v) = AC(u, v
(u, v) e X x N(B).
(37.*)
(II) Solution of(37.*) via the Leray-Schauder principle (continuation \vith
respect to i.). We will show below that the following two conditions are
satisfied:
(i) The operator C: X x N(B) -. X x N(B) is compact.
(ii) There is a number k > 0 such that
lIuli + IIvll s k
for all solutions (u, v) of (37.*) with 0 < A. < 1.
Then the Leray Schauder principle (Theorem 6.A) tells us that equa-
tion (37**) has a solution for A = I, i.e., the original equation (33) has
a solution.
(11-1) We prove (i). The operator N: X -+ X is compact and S-I: X -+ X is
linear and continuous. This yields (i). Note that dim N(B) < 00, and
hence continuous bounded operators on N(B) are compact.
(11-2) We prove (ii), Since dim N(B) < 00, the set Y = {v e N(B): II vB = I} is
compact. By (34
It r min [a(v) - (vlb)] > O.
I.' CI r
By (H2),
sup{Ug(u)lI: u E X} < 00.
Thus it follows from (H3) that
I , Ir(v,g(u»1 0
1m sup = .
1I&11'H;X II vII
(38)
Again by (H3),
p dCr (vIN(v + 9(11» - b) = a(v) - (vlb) + r(v,g(u»
Illlvn+r(v,g(u» Corall veN(B), ueX, (39)
Now let (u. v) be a solution of (37.). By the second equation of (37*),
II vII 2 = A 2 ( UVU2 - 2,P + ,2 t. (If )
i-I
< l2(lIv2 - 2tp(lIvn + o(nvll) + t20(1) IIvll ... 00. (40)

29.9. Application to Semilinear Elliptic Differential Equations
661
Note that SUPi locil < 00. By (40 there is a number k l such that
IIvll < k l
for all solutions (u, v) of (37.) \\'ith 0 < A < I. Otherwise, there is a
sequence (u II , v,,) of solutions of (37*) with II V n II ..... OCt as n ..... co. Letting
v = v" in (40) and dividing by II villi 2, we obtain 1 S A, Z as II -+ X). This is
a contradiction.
By the first equation of(37*), there is a number k 2 such that lIuli < k 2
for aU solutions (u, v) of (37.) with 0 < ,t < 1, by (38).
Ad(b). Let Bu + Nu = b. Suppose that
(vi N(v + ,,,.» < a(v) for all v e N(B) - {O}, 'v E X.
The operator B is self-adjoint. From (vIBu) = (Br4Iv) = 0 for all v E N(B) it
follows that
(vi Nu) = (blv).
Hence (blv) < a(v) for all t' E N(B) - {O}.
o
Replacing t by - t, we obtain Corollary 29.7.
29.9. Application to Semilinear Elliptic Differential
Equations of the Landesman-Lazer Type
We want to apply Theorem 29.D to the boundary value problem
-411 - JlU + h(u) = f on G,
u = 0 on oG,
\vhere Jl is a fixed real number. The corresponding linearized problem reads
as follows:
(41)
-Au - /JU = 0 on G..
u = 0 on aGe
If Jl is not an eigenvalue of (42) (resp. if Jl is an eigenvalue of (42», then we
speak or the nonresonance case (resp. resonance case). We assume:
(42)
(H 1) G is a bounded region in frI, n  1.
(H2) The function h: (R ..... (Ii is continuous and bounded.
(H3) There exist the finite limits
h( :too) = lim h(s)
s- :t
and IJ( -oc.) < h(s) < h( +00) for all s e IR (cr. Fig. 29.3 above).

662
29. Noncoerave Equations and Nonlinear Fredholm Alternatives
Let liE W2'(G), m > 1. We set
G 1 = {x e G: u(x)  O}
and
at (II) = f. h( + OO)II(x)dx + f. h( + oo)u(x)dx
G. G_
for u :F O. In the case where II = 0 let a(O) = O. As usual, we set IM. . . dx = 0
if M = 0. Note that a f is well defined. Indeed, if we change u on a set of
measure zero, then G! may change, but Q:t(u) remains unchanged.
We first formulate a simple necessary solvability condition for (41) in the
resonance case. To this end, let (p, "0) be a classical eigensolution of (42) and
suppose that the original problem (41) has a classical solution. Using inte-
gration by parts, it follows from (41) that
fG Juo dx = fG h(uo)u o dx.
By (H3), \ve obtain
a_(uo) < fG uoJ dx < a.(uo). (43)
In Proposition 29.10 below we will obtain the sr,rprising fact that the simple
necessary solvability condition (43) for problem (41) is also sufficient in the
case where the eigenvalue J.l is simple.
Instead of (41) we consider the more general problem
Lu - 1JU + h(u) = f on G,
D"'u = 0 on aG
together with the linearized problem
Lrl - pll = 0 on G,
for all y: II'I S m - I,
(44)
D"'u = 0 on oG
for all /: I}'I  m - I,
(45)
where m  I and
Lu(x) = L (-I)IDar(a«_(x)D"u(x».
1-1.11'1 s '"
(H4) Let the functions aar,: G -t R be measurable and bounded with atJI = al tl
for all IX, (J. Suppose that L is regularly strongly elliptic or strongly elliptic
in the sense of Definition 22.42.
Note that (41) is a special case of (44) with m = 1.
Definition 29.9. Let X = W 2 "'(G). The generalized problem for (44) reads as
follows. For given f e L 2 (G), we seek u E X such that
c(u, v) + d(u, v) = b(v) for all VEX. (46)

29.9. Application to Semilincar Elliptic Diff'crential Equations
663
Here we set
c(u, v) = J. ( L a«,D-u[)2v - /J UV ) dX,
G tJl.I'1 S '"
d(u, v) = J. h(u)v dx, b(v) = J. Iv dx.
G G
Formally, we obtain (46) by multiplying (44) with v e Ct(G) and using
subsequent integration by parts.
In the special case (41), we obtain
c(u, v) = J. ( f DjuDjv - J.luv ) dx,
G ;:.1
where x = (I'. .., ") and D, = o/c,.
Proposition 29.10 (Landesman and Lazer (1969». Assume (HI) through (H4).
Tllen:
(a) The non resonance case. If JJ is not all eigenvalue of the generalized problem
for (45), tl,en for each Ie L 2 (G), the generalized problem for (44) lias a
501111 ion.
(b) The resonance case. If p is a simple eigenvalue of Ihe generalized problem
for (45) \vith the eigenfunction u o , then the generalized problem for (44) laas
a solution iff condition (43) is satisfied.
Corollary 29.11. Statement (a) remains true if L is not symmetric and assrllnption
(H3) drops out.
PROOF. Ad(a). We apply Theorem 29.C. Using the Identification Principle
21.18, we set X = X*. We define the operators B, N: X -+ X through
(Blllv)x = c(u, v), (Nulv)x = d(u, v) for all u, VEX.
By (22.lb b e X*. Hence the generalized problem (46) is equivalent to the
operator equation
Bu + Nu = b,
u eX.
(47)
It follows from (H4) and Proposition 22.45 that c(., .) is a regular Garding
form. By Proposition 21.31 and Lemma 2238, B is the sum of a linear strongly
monotone operator and a linear compact operator. Hence it follows from
Theorem 21.F that B is Fredholm of index zero. By Figure 27.1, B satisfics
condition (S).
By Corollary 26.14, the operator N: X -+ X is strongly continuous. Since h
is bounded,
I(Nulv)1 = fG h(u)vdx S const(fG V 1 dX)H2
S const IIvllx for all u, t' e X,
i.e., II Null S const for all u eX.

664
29. Noncoerci,'c Equations and Nonlinear Fredholm Altemati\'e$
For each t e [0, 1], the operator U'-' Bu + t(Nu - b) is a strongly continu-
ous perturbation of the (S)-operator B. Thus, this operator also satisfies
condition (S), by Figure 27.1.
Since Jl is not an eigenvalue of (45) Bu = 0 implies u = O. Now, statement
(a) and Corollary 29.11 follow from Theorem 29.C.
Ad(b). We apply Theorem 29.D. By assumption, dim N(B) = I and
N(B) = { + tuo: I  O}.
Since aa.6 = Q6a.' we obtain c(u, v) = c(v, u) Cor all u, veX, i.e., B is self-adjoint.
The operator B is Fredholm. Hence R(B) is closed.
(I) We show that N has weak asymptotes on N(B). By (H2) the principle
of majorized convergence A 2 ( 19) yields the ke}1 relation:
lim (N(lUo + \v)luo) = lim f. h(tuo + "')11odx
,... ,"'+oc G
= f. h( +oo)uod.;t + f. h( -oc)uodx = a+(uo).
G. G
(48)
This convergence is uniform with respect to \v on each bounded subset
of X. Otherwise, there are a number t > 0, a bounded sequence ("'II) in
X.. and a real sequence (t.) with tft -. +00 as II -+ 00 such that
I(N(t"r1o + wn)lu o ) - a+(uo)1 > e for all n e N. (49)
Since the embedding J-tt 2 -(G) S L 2 (G) is compact, we may assume that
\\.... -. "r in Lz(G) as n --+ 00. This implies
,v..(x)  w(x) as n --.. 00 Cor almost all x E G.
Using majorized convergence. it follows from (49) that la+(uo)-
a+(uo)1  t. This is a contradiction.
We set
( ) { ,a+(uo)
a tu o = 0
if t  0,
if t = o.
Relation (48) and a similar relation as t --+ -co imply
(IV(tu o + ,,')Ituo) = a(tuo) + r(tuo, ",),
Cor all t e R, where
I . Ir(trlo,v)1 0
1m sup = ,
'''':!:(I) WJ: III
for each bounded subset K of X. This is relation (35).
(II) We sho\v that
(N(t, + \\')Iv) < a(v)
for all ve N(B) - {O}. \\' e X.

29.10. The Main Theorem on Nonlinear Proper Fredholm Operators
665
This follows from
(N(IUo + w)IIUo) = fG h(IUo + w)luodx < ra%(uo) if I  0,
since h( - 00) < II(s) < h( + 00) for all s e (R.
(III) By Theorem 29.D, the condition
b(v) < a(v) for all v E N(B) - to} (50)
is necessary and sufficient for the so(\'ability of the equation Bu + N u =
b. U EX. Condition (SO) means
fG IUof dx < la:t(uo) if I  o.
This is the solvability condition (43). 0
29.10. The Main Theorem on Nonlinear
Proper Fredholm Operators
Let nx(b) denote the number of solution.,; of the equation
Au = b,
lIeX.
(51)
We want to study the properties of the function nx(.) on the image space Y
of the operator A. We set
D sin . = {u e X: A'(u)h = 0 has a solution h #: O},
i.e., D sina is the set of singular points of A.
Theorem 19.E (Main Theorem). Let X and Y be B-spaces over K = R, C. and
let A: X -. Y be a proper C'-Fredholm operator of index zero ,,'it/l I S k S
00. Then:
(a) For each bEY - A(DAina the number nx(b)  0 isfinile.
(b) Tile function nx(.) is COllstant on each con"ected subset of the open and
dense subset Y - A(D'in.) of 1':
(c) For each u in tile open set X - DS'DI' tile operator A is locally invertible,
i.e., A is a local C-diffeomorphism at u.
(d) If the set D. in . is empty, tllen A: X --. Y ;s a CA-difJeomorphism.
(e) The set A (D ,1D ,) of singular values of A is closed and no "'here dense in Y.
The proof of Theorem 29.E win be given in Section 29.IOe after a detailed
discussion of Theorem 29.E.
Recall that (c) is equivalent to the following important fact. Let u e X -
D l1na be a solution of the original equation (51). Then there arc neighborhoods

666
29. Noncoerci\'c Equations and Nonlinear Fredholm Alternatives
U and V of u and b, respectively, such that for each b e V the equation
A u = b, U E U.
has a unique solution u . If we set u = B(b then the solution operator B is C A
on V.
The fundamental Theorem 29.E tells us that proper Fredholm mappings of
index zero behave like "reasonable n functions A: R -.(R (Fig. 29.4(a), (b».
Roughly speaking, we obtain that, for "almost aU" right members h, equation
(51) has at most a finite number of solutions, and this number may bifurcate
only at the singular values b E A(D. in .). This critical set A(D s ..,,) is nowhere
dense in Y, i.e., it is meagre.
The more general equation Au = b, u e S, where S is an arbitrary subset of
the B-space X, will be considered in Theorem 29.F below.
EXAMPLE 29.12. The CI-function
A:R....R
satisfies the assumptions of Theorem 29.E iff
(52)
IAul .... oc:
as I ul .... 00.
(53)
Moreover, we have
U E D'I'"
iff
A'(u) = O.
b
p- -
Q--
D,,.
(a) proper
(b) proper
----b
(c) not proper
Figure 29.4

29.10. The Main Theorem OD Nonlinear Proper Fredholm Operators
667
The function A in (52) is always Fredholm of index zero. The condition (53)
is equivalent to the properness of A.
In Figure 29.4 (a) the set D sin . consists of the two points p and qt and the
set A(D sin .) of the singular values of A consists of the two points P and Q. The
function nR( · ) bifurcates at P and Q. Figure 29.4(a) allows an intuitive interpre-
tation of Theorem 29.E.
Figure 29.4(b) shows that, in contrast to A(D 5In .), the set D81'" is not always
meagre.
If A is not proper. i.e., the growth condition (53) is violated, then Theorem
29.E is not always true. Look for an example in Figure 29.4(c) which shows
Au = sin u. Thus, the assumption of properness is essential in Theorem 29.E.
PROOF. This follows easily from the definition of the notions "proper" and
"Fredholm operator" given in Part It which we recall in what follows. 0
29.10a. Basic Definitions
Recall from Section 4.16 that a mapping is called proper iff the preimages of
compact sets are again compact. If the operator A: D(A) e X..... Y is con-
tinuous, where X and Yare B-spaces, then A is proper iff:
Au" ..... a as n -. C1J implies the existence of a subsequence u". -. u as n .... 00
such that u e D(A).
Recall from Section 8.4 the following. Let X and Y be B-spaces over D< = R,
C, and let L(X, Y) denote the set of all linear continuous operators C: X -+ 1':
Then. L(X, Y) is a B-space over I< with respect to the operator nonn. The
linear operator
c: X -+ y
is called Fredllolm iff C is continuous and
dim N(C) < co and codim R(C) < 00.
where N(C) = {u e X: Cu = O} and R(C) = C(X). This implies that the range
R(C) is closed. The index of C is defined through
ind C = dim N(C) - codim R(C).
The number dim R(C) is called the r(lnk of C.
The nonli'lear operator
A: D(A) s; X -+ Y (54)
on the open set D(A) is called Fredholm iff A is C. and the linearization, i.e.,
the F-derivative
A'(u): X -+ y
is Fredholm for all u e D(A). The number dim R(A'(u» is called the rank of A

668
29. Noncoercive Equations and Nonlinear Fredholm Alternatives
at u. Furthermore, the number ind A'(u) is called the index of A at u. If D(A)
is connected, then the index of A is constant on D(A), by (56) below. If A in
(54) is a Fredholm operator with ind A'(u) = const. = m on D(A), then A is
called a Fredholm operator of index  and we write ind A = m. For example,
the CI-operator A in (54) is Fredholm of index zero iff
dim N(A'(u») = codim R(A'(u» < 00 for all U E D(A). (55)
Let A: D(A) e X.... Y be Fredholm on the open set D(A). The point II is
called a singillar poi'Jt of A iff A'(u): X -. Y is not surjective. Otherwise, u is
called a regular point of A. Furthermore, the point bEY is called a si'Jgular
t'aille of A iff there is a singular point II of A such that Au = b. Otherwise, b
is called a reglliar vallie of A. In particular, if A is Fredholm of index zero, then
u is a singular point of A iff the equation A'(u)1J = 0 has a solution h =1= o.
Singular points (resp. singular values) are also called critical points (resp.
critical values).
Proper Fredholm operators playa fundamental role for the following t\\'O
reasons:
(i) Localization. Fredholm mappings renect the fact that the linearizations of
large classes of nonlinear integral equations and differential equations
have the following two important properties:
The linearized homogeneous problem lias 0111)' a finite nllmber of linear/}'
independent solutiolls (dim N(A'(II») < 00).
The li'Jearized inhomogeneorls problem lIas onl)' a/illite number of linear/}'
independent soltVlbilit)' cOllditiolls (codim R(A'(u) < 00).
(ii) Globalization. In order to globalize local results one uses proper maps.
29. lOb. Typical Properties of Linear Fredholm Operators
Summary 19.13. Let C, D E L(X, Y), wllere X a"d Yare B-spaces over I< = It
C. The fo 110 \ving properties are filndamental:
(a) IfC is f-redholln and liD II is sufficiently smal" t',en the perturbation C + D
is also Fredholm and we 'Jave:
ind(C + D) = indC
(56)
alld
rank C  rank(C + D),
codim R(q  codim R(C + D),
dim N(C)  dim N(C + D).
That is, the set !F oJ'li'Jear Fredholm operators from X to Y is open ill
L(X, Y), and tile index is locally constant and he'lce cOlltinuous Oil !F.

29.10. The Main Theorem on Nonlinear Proper Fredholm Operarors
669
Moreover, the rank ;s lo\ver semiconti"uous on. I:inall}', the codilnenS;OIl
of tile range and the dimension of the null space are upper senl;colltinuous
on.
(b) If C is Fredholm and D is co'''pacl, then C + D is also Fredllolm and
ind(C + D) = ind C.
(c) Let C be Fredholln of index zero. Theil C: X -. Y is a Coo-diffeomorphisln
iff Ch = 0 ilJlplies h = O.
(d) Let C be Fredholm of negatilJe i"dex. Theil C: X -t R(C) is a Cl:TJ-diffeo-
Inorphism iff CII = 0 implies h = O.
(e) A Fredholm operator C: X -. Y of negative (resp. positive) index call lIever
be surjective (resp. ilJjective).
(f) If dim X < 00 and dim Y < 00, then each linear operator C: X -. Y is
Fredholnl and ind C = dim X - dim Y.
Statement (c) underlines the importance of Fredholm operators of index zero.
From (f) it follows that Fredholm operators bet\\'een B-spaces are a natural
generalization of linear operators between linite-dimensionallinear spaces.
Further important properties of linear Fredholm operators and references
to the relevant literature can be found in Section 8.4.
29.1Oc. Typical Examples
\Vc consider two typical situations.
EXAMPLE 29.14 (Finite-Dimensional Spaces). Let X and Y be B-spaces over
K = R, C with
dim X = dim Y < 00
(e.g., X = Y = iii". N > 1). Let A: D(A) e X... Y be a C'-map on the open set
D(A). Then:
(a) A is Fredholm of index zero.
(b) Let D(A) = X. Then, A is proper iff A is weakly coercive, i.c.,
IIAull -+ 00 as lIu II -+ oc. (57)
(c) Let D(A) be bounded. Then A is proper iff
II Au" -+ 00 as dist(u, oD(A» -. O. (58)
(d) A is proper on each compact subset of D(A).
PROOF. Cf. Example 4.42. 0
In the case where X = Y = R, Figure 29.S shows examples for proper maps,
whereas the functions pictured in Figure 29.6 are not proper.

670
29. Noncocrcive Equations and Nonlinear Fredholm Alternatives
D(A)
(a)
(b) D(A) = R
(c) D(A) = R
Figure 29.5
)
/
D(A)
(

- .
--------
(a)
(b)
Figure 29.6
STANDARD EXAMPLE 29.15 (Compact Perturbations of Diffeomorphisms). Let
X and Y be B-spaces over II( = A, C. Suppose that:
(i) The map B: X  Y is a C 1 -diffeomorphism.
(ii) The map C: X --. Y is compact and C 1 .
(iii) The map A = B + C is weakly coercive, i.e., IIAull -. 00 as lIuli  00.
Then, A: X  Y is a proper C 1 -Fredholm operator of index zero i.e., Theorem
29.E can be applied to the operator A.
PROOF. This is a special case of the following Example 29.16.
o
EXAMPLE 29.16 (Compact Perturbations of Local Diffeomorphisms). Let X
and Y be B-spaces over k =  C. Consider the map
A: D(A)  X  Y with A = B + C
on the open set D(A). Suppose that:
(i) The C 1 -map B: D(A)  X -to Y is a local diffeomorphism at each point of

29.10. The Main Theorem on Nonlinear Proper Fredholm Operators
671
D(A). By the inverse mapping theorem, this is equivalent to the fact that
B'(u): X .... Y is bijective for all" E D(A).
(ii) The CI-map C: D(A) c X -+ Y is compact.
Then:
(a) A is Fredholm of index zero.
(b) Let B be proper (e.g., B is bijective) and suppose that for each bounded
set M in  the set A -I (M) is bounded and if, in addition, D(A) #= X t then
dist(A- 1 (M), cD(A» > O. Then, A is proper.
(c) Suppose that B is injective and the set B(N) is closed for each
closed bounded subset N of D(A) with the additional property that
dist(N,oD(A» > 0 if D(A) :#: X. Suppose that the operator A has the same
properties as in (b). Then, A is proper.
(d) A is proper on each compact subset of D(A).
PROOF. Ad(a). By (i), Ir(u) is bijective and hence B'(u) is Fredholm of index
zero. By Proposition 7.33, the compactness of C implies the compactness of
C'(u). Thus A'(u) = Ir(u) + C'(u) is Fredholm of index zero, by Summary
29.13(b).
Ad(b). Let M be compact, and let (u ll ) be a sequence in A-I (M). We have
to show that there exists a subsequence Un' -. u as n -+ cc such that u E A-I (M).
Since A is continuous and M is compact it suffices to show that u e D(A).
Since (u.) is bounded and the operator C is compact, there exists a sub-
sequence, again denoted by (un such that CUll -. C as n -. 00 for some c. Since
the set M is compact, there is a subsequence, again denoted by (u lI ), such that
Au. -+ a as n -+ ex; for some Q. Hence
Bu = Au - Cu -. a - c
" . n
as n -+ XJ.
Since B is proper, the set {u.: n eN} is relatively compact. Thus there is a
subsequence, again denoted by (u II ), such that Un .... U as n .... 00 for some u.
From dist(A -I (J\t), eJD(A) > 0 we obtain that u e D(A).
Ad (c). As in the proof of (b) we obtain that BUn -t a - cas n -t 00. Let N be
the closure of the bounded set {u II : II EN}. Then dist(N, oD(A» > 0, and hence
B(N) is closed. Consequently, a - c e R(B). Since B is locally invertible and
injective, we get Un .... U as n -. CX) where u = B-1(a - c). Hence U E D(A).
Ad(d). We consider the operator A: N -+ t: where N is a compact subset of
D(A). Let M be a compact subset of Y. Since A is continuous, the set A -I (M)
is a closed subset of the compact set N, and hence A-I (M) is compact
(cf. Al ( 11 ) ). 0
29.1Od. Typical Properties of Nonlinear Fredholm Operators
The following proposition collects fundamental properties of nonlinear
Fredholm operators.

672
29. Noncocrcivc Equations and Nonlinear Fredholm Altcmati,'cs
Proposition 29.17. [.let X and Y be B-spaces OL'er IK = R, C, and let
A: D(A) e X...... y
be a Ck-Fredholm operator OIl the open set D(A), \\'here I  k  00. TheIl:
(a) Compact perturbations. If C: D(A) e X... Y is compact and C 1 , tllen
A + C is a Fredholln operator ,,'itll the same index as A at each POillt of
D(A).
(b) Stability of the index. Let 'I be a cOllnected subset of D(A). Then,
ind A'(v) = const for all v e tt'.
(c) Lower semicontinuity of the rank. For each u e D(A there exists a
neighborhood U of u such that, for all t' e U:
rank A'(u)  rank A'(v).
codim R(A'(u) > codim R(A'(v»),
dim N(A'(u»  dim N(A'(v».
Recall that rank A/(u) = dim R(A'(u».
(d) Local closedness. The lnap A ;s locally closed, i.e.,for each u e D(A), tlJere
exists a neighborhood U such that A nlaps ever)' closed subset of U onto
a closed set.
(e) Regular points. The set of regular points of A is open. If u is a regt41ar point
of A, then tile inde. of A at u is nonnegative.
(f) Regular values (Sard-Smale theorem). Let
k > max(ind A'(u), 0) for all u e D(A), (59)
and let X and Y be separable. Tllen ti,e set of singular f1Vllues of A is 1I0,,'lJere
dense alulilence of first Baire category in Y (i.e., this set is meagre), \\fhereas
the set of regular values of A is dense and of second Baire category in Y
(i.e., this set is "big").
(g) Regular values or proper maps. Suppose that (59) holds and tllat A is proper.
Then the set of singular values of A is nowhere dense and hence of first Baire
categor}' ill Y, \\'hereas the set of regular values of A is open and dense and
hence of second Baire category in Y.
(h) Preimage theorem. If b is a regular value of A, thell the solution set M of
the equat;oll
Au = b,
u e D(A),
(60)
;s a C" -sublnanifold of X.
The dimension of M at each point u is equal to dim N(A'(u).lf dim X <
oc and dim Y < 00, then
dim JW = dim X - dim y:
(i) Local diffeomorphism. Suppose that A has index zero at u. Then A is a
local C"-diffeonlorphism at 14 iff A'(u)h = 0 implies h = O.

29.10. The Main Theorem on Nonlinear Proper Fredholm Operators
673
(j) Well-posedness of equation (60). If A has index zero and b o is a regular
.'alue of A, chen ho e int R(A), i.e., the equation (60) has a soilltionfor all b
in a neighborhood of b o .
(k) Ill-posedness of equation (60). Suppose that (59) Iioids and chat X and Yare
separable. If A lias a negative index at each point of D(A) then int R(A) =
0, i.e., if equation (60) has a soilition/or b = b o , tl,en in each neighborhood
0.( b o , tllere ;s a b sIIclalhat (60) has no solution.
The reader should check that all the statements above can be easily verified
in the case where the operator A: X ..... Y is linear with X = R" and Y = !R"'.
Here, ind A = " - m. Statement (i) above underlines the importance of
Fredholm operators of index zero.
The deep results of Proposition 29.17 are contained in (f) and (8). These
statements are special cases of the Sard -Smale theorem on Banach manifolds
\vhich will be proved in Section 78.9 of Part IV. There we will also give the
simple proof for (d). In the proof of Theorem 29.E below, we only need (e), (g).
and (i).
PROOF. Ad(a). Since C is compact, so is C(u). The theory of linear Fredholm
operators tells us that the compact perturbation A'(u) + C'(u) of the Fredholm
operator A'(,,) is again a Fredholm operator with the same index as A'(II).
Ad(b). Set feu) = ind A'(u). By (56), the function f: D(A) -. R is locally
constant and hence continuous. Thus,f( is connected, since <6 is connected.
Ho\vever, f is integer-valued and hence f is constant on fG.
Ad(c). This follows from (56).
Ad(d). cr. Section 78.9.
Ad(e). The point u is regular iff
codim R(A'(u» = O.
This implies ind A'(r,) = dim N(A'(u»  O. By (c), there is a neighborhood U
of rl such that
codim R(A'(v)  codim R(A'(u» = 0
for all v e U, i.e., all the points v in U are regular.
Ad(f), (g). Cf. Section 78.9.
Ad(h). Theorem 4. J tells us that the solution set of (60) is aCt-Banach
manifold. The fact that this set is indeed a submanifold of X will be proved
in Section 73.11.
Ad(i). Since A'(rl) has index zero, this operator is bijective iff A'(u)1a = 0
implies h = O. Thus, the assertion follows from the inverse mapping theorem
(Theorem 4. F).
Ad(j). This follows from (i).
Ad(k). Suppose that int R(A) :I: 0. By the Sard-Smale theorem (0, there is
a regular \'alue b in R(A), i.e., there is a u such that A'(u) is surjective. But this
is impossible, since the index of A'(II) is negative. 0

674
29. Noncoerci\'c Equations and Nonlinear Fredholm Alternati\'eS
29.10e. Proof of Theorem 29.E
(I) By Proposition 29.17(e), the set X - D'ina of the regular points of A is
open. If u e X - D tiOI ' then A is a local diffeomorphism at u, according
to Proposition 29.17(i).
(II) Let bEY - A(D sioa )' Since A is proper, the set A -1 (b) is compact. By
(I), the points of A-I(b) are isolated. Hence the set A-1(b) is finite.
(III) We show that nx(') is constant on some neighborhood of b.
Since DIAD' is closed and A is proper, the set A(D I6D ,) is closed, by
Proposition 4.44.
(111-1) Let A- 1 (b) = 0. Hence nx(b) = O.lfthe assertion (III) is not true, then
there exists a sequence (VII) such that
Av" ... b
as n  OC>,
and hence nx(Av,,) > O. Since A is proper, there exists a subsequence,
again denoted by (I'.)' such that
v"  v
as n... 00.
Hence Av == b. This contradicts A-I(b) = 0.
(111-2) Let A -I (b) = {" I'" ., u,}. Since u, E X - D S'nl for all i and the set
X - D i1nl is open, there exist pairwise disjoint sufficiently small open
neighborhoods VI' ..., Vie of "I' ..., u" respectively, such that
A: Vi -+ A(V,)
is a Ck-difTeomorphism, where A(V,) is a neighborhood of b for each i.
Set
t
U = U Vi'
i1
If nx(') is not constant on some neighborhood of b, then there exists
a sequence (b,,) with
bit -+ b
as n.... 00
and nx(b,,) > k for all n. Consequently, there is a sequence (VII) with
AV II = b ll and v. e X - U for all n.
Since A is proper, there exists a subsequence. again denoted by (v,,),
such that VII -+ V as n -+ 00 and hence
Av = b,
veX - V,
i.e., V #: u, for all i. This is a contradiction.
(IV) Let <6 be a connected subset of Y - A(D sinl )' We show that nx(') is
constant on lI. In fact, it follows from (111) that the function nx: <G -+ R
is continuous. Hence nx() is connected. Since "x is integer-valued, this
function is constant on fG.

29.10. The Main Theorem on Nonlinear Proper Fredholm Operators
675
(V) By the Sard-Smale theorem (Proposition 29.17(g», the set Y-
A(D sin .) is open and dense, and A(D,inl) is nowhere dense in 1':
(VI) Let D sina = 0. Since the proper map A: X -+ Y is a local -
diffeomorphism at each point of X, it follows from the global inverse
mapping theorem (Theorem 4.G) that A is act-diffeomorphism.
The proof of Theorem 29.E is complete. 0
29. 1 Of. Generalization to Arbitrary Sets
We now want to study the number of solutions ns(b) of the equation
Au=b, ueS, (61)
where S is an arbitrar)' set in a B-space. To this end, we set
D Sinl = {u e int S: A'(u)h = 0 has a solution h =F O}.
We define the set Y erAe of the critical right members b of equation (61) through
ril = A (D sinl ) U A (as "S),
and we set e. = Y - ,it.
The following theorem is fundamental:
Theorem 29.F (Solution set of(61»). Let X and Y be B-spaces ofl'er IK = R, C.
Suppose that:
(i) Tile map A: S S X -. Y is proper and continrlous.
(ii) The map A: int S -+ Y is C" and Fredholm of index zero. 1 S k S 00.
Then:
(a) F or each point u in the open set int S - D'ins' the map A is a local C*-
diffeomorphism at u.
(b) Let b E ea. Then the number ns(b) > 0 is finite. Moreover, the map A is a
local Ct.diffeomorphism at each point u of the solution set of equation (61).
(c) The filnction ns( · ) is constant on each connected subset of the open set el.
(d) If A is proper on int S, then the set
CI u A(aS n S)
is opelJ and dense in Y and hence 01 second Haire calegor}' in Y, and the
set A(D'iDI> is nowhere dense in 
(e) If X and Y are separable, then the set CI u A(oS "S) is dense and of second
Baire categor}' in  and the set A(D.. n .) is nowhere dense in Y.
(f) If A is injective on int S - D ainl , then the map
A: iot S - D"na -+ A(int S - D'in.)
is a C"-diffeomorphism.
(g) lIS = X and D"n. = 0. then A: X -+ Y is a C"-diffeomorphism.

676
29. Noncoercive Equations and Nonlinear ....redholm Altemati,'cs
R
b 2 R
P
hi P
Q b
ho
T Q
- I .
\
S
.---A
\
s
.
(a) "s (b o ) == 2. "s (b I) = 3, liS (h 2 ) = I
(b)
Figure 29.7
This theorem tells us that the function ,Js( · ) may only bifurcate at the points
of the critical set Y eri .. Up to possible pathological situations, the set A(o S f'a S)
is "thjn and hence the set rit is also "thin" and the set ea is "big", according
to (d) and (c). In connection with Example 29.19 below, simple counter-
examples for X = R show that Theorem 29.F is '6sharp.
In Section 29.1Oc we have proved the properness of maps A: D(A) S X -+ 
",'here D(A) is open (e.g., D(A) = X). The following corollary shows how to
use this infonnation in order to prove the properness of A on closed sets S.
Corollary 29.18 (Properness). Lee X and Y be B-spaces ot:er [K = R, C. Then:
(a) If A: D(A)  X -+ Y is proper, then A: S C X -t Y is proper on each closed
subset S of D(A).
(b) If S is compact, thell each continuous nJap A: S c X --. Y is proper.
This follows from the fact that closed subsets of compact sets are compact.
EXAfPLE 29.19. Figure 29.7 illustrates the intuitive meaning of Theorem 29.F.
The number Ils(b) of solutions of the equation Au = b, b E S, may only
bifurcate at the points P, Q, R, T. Here, we have
rlt = {P, Q, R, T} and el = Ii - Y efit .
In Figure 29.7(a) we obtain that
A(aS ra S) = {Q,R} and A(D sina ) = {P, T},
and in Figure 29.7(b) we have A(oS r. S) = {Q} and A(D sin .) = {P, R}.
PROOF OF THEOREM 29.F. Ad(a). This follows from Proposition 29.17(e (i).
Ad(b). Let b e c8. Then b fI A(eS n S) U A(D sin .) and hence
A -1 (b) c int S - D sID ,.. (62)
Since the map A is proper, the set A -I (b) is compact. By (a), all the points of
A-1(b) are isolated. Hence A-1(b) is finite.

29.11. LocaJly Stricti)' Monotone Operators
677
Ad(c). We first prove that the set e. is open. In fact, by (a) the set
So = int S - Dr-IRa is open in X. Thus, the set So is also open with respect to
the induced topology on S, and hence the set
(as tl S) U D 1inl = S - So
is closed \vith respect to the induced topology on S (cf. AI (8». Since A: S -+ Y
is continuous and proper with respect to the topology on X, this map has the
same properties with respect to the induced topology on S. Therefore, by
Proposition 4.44, the set Y erit = A(S - So) is closed in  and hence ea = y -
ril is open in Y.
The following simple observation is crucial. Let
Av" = bll'
v" e S
for all n
and bIt ..... b as II --+ 00. Since A is proper, the sequence (VII) lies in a compact
subset of S. Thus_ there exists a subsequence, again denoted by (VII)' such that
v" -+ l' as n -+ 00 and v e S. Since A is continuous,
Av = b.
Using this observation, the proof of (c) now proceeds as the corresponding
proof of Theorem 29.E in Section 29.10e. In this connection, observe that
b e e. implies (62) above.
Ad(d), (e). The set of regular values of the map A: int S ..... Y is equal to
y - A(Dina) = c. U A(oS ("'\ 5).
follows from the Sard-Smalc theorem (Proposition
Now the assertion
29.17(f), (g».
Ad(f). This follows from (a).
Ad(g). This follows from Theorem 29.E.
o
In the follo\\'ing sections we will consider applications of Theorem 29.F.
29.11. Locally Strictly Monotone Operators
Definition 29.20. Let X be a real B-space. The operator A: D(A) c: X -+ X. is
called 10 ca II}' strictly monotone at the point u iff u e int D(A) and the F-
derivative A'(u): X -. X* is strictly monotone, i.e.,
(A'(u)h, h> > 0
for all heX with h -I: O.
(63)
The operator A is called locally strictly monotone on the set S iff it has this
property at each point of S.
The operator A is called locall)' strongl}1 monotolle at u iff u e int D(A) and
the F-deri\'ative A'(u) is strongly monotone, i.e., there is a c > 0 such that
(A'(u)h. h)  ell hll 2
for all heX.
(64)

678
29. Noncoerci\'e Equalions and Nonlinear Fredholm Alternatives
A

u
Figure 29.8
Analogously, the operator A is called locall)' monotone at u iff u e int D(A)
and the F-derivative A'(u) is monotone.
In this connection, we assume tacitly that the F-derivative A'(u) exists.
In Section 29.12, we will also introduce the notion of locally regularly
monotone operators which playa fundamental role in the calculus of varia-
tions for obtaining sufficient conditions for strict local minima. If the B-space
X is finite dimensional, then the following three notions coincide: locally
strictly monotone, locally strongly monotone, and locally regularly monotone.
EXAMPLE 29.21. The function A: D(A) s; IR -+ R is locally strictly monotone at
the point u iff u e int D(A) and
A'(u) > O.
Thus, if A is locally strictly monotone on the open interval U = ]a, b[, then
the real function A(.) is strictly monotone increasing on U (Fig. 29.8). The
following results generalize the behavior of such functions.
We first investigate the connection between locally monotone operators
and monotone operators.
Proposition 29.22. Let A: U c: X -+ (R be C. on the open subset U of the real
B-space X, and let C be a cont'ex subset of U. Then:
(a) If A is loeall)' strictly monotone on C, then A is stricti}' monotone on C.
(b) If A is locall)' monotone on C, then A ;s nlonotone on C.
PROOF. Ad(a). Let u, v e C with u :/: v. Set II = V - u. By Taylor.s theorem
(Theorem 4.A),
Av - Au = LI A'(u + th)h dt
and hence
(Av - Au. v - u) = JOI (A'(u + th)/., h) dt > O.
Note that the integrand is positive for each t e [0, 1], since A is locally strictly
monotone on the convex set C.
Ad(b). Use the same argument. 0

29.12, Locally Regularl)' Monotone Operators, Minim and Stability
679
Proposition 29.23 (Local DifTeomorphisms). Let A: U e X..... X. be a ct.
Fredl.olm operator o..f inde.'( zero 0" tile ope" subset U of tIle real B.space X,
and let A be locall} stricti}' monotone on U, where I  k S oc. Then:
(a) Tile map A is a local C".diffeomorpllis", at eacll point of U.
(b) If U ;s contex. tllen A: U  A(U);s a strictly monotone C*-diffeomorpl,is",.
PROOF. Ad(a). If A'(u)II = 0, then II = 0, by (63). Thus, the assertion follows
from Proposition 29.17(i).
Ad(b). By (a), it is sufficient to prove that A is injective. But this follows from
the strict monotonicity of A, according to Proposition 29.22. 0
We now study the "",,,ber o..f solutions ns(b) of the equation
Au = b..
u e S.
(65)
Theorem 29.G (Benkert (1985). Let S be a subset oftlJe real B-space X. Suppose
tllat:
(i) TI,e map A: S c X -. X. is proper alul cont;nuorls.
(ii) The ",ap A: int S -+ X. ;s a C'-Fredhol"l operator of index zero and locall}'
str;cll) nlo"otone 011 int S, \vhere I < k < 00.
TllelJ:
(a) For eacla b e X. - A(t'S ('\ S), ti,e number ns(b) > 0 is finite.
(b) The functiolJ ns(.) is c01l.'itant OIl eacll conlJected subset of the ope" set
X. - A(oS" S).
(c) III the case ,,,here S = X, tlae map A: X -. X. is a C'-dijJeonaorphism.
This result is related to the main theorem on monotone operators
(Theorem 26.A).
PROOF. This is a special case of Theorem 29.F. Note that A'(u)It = 0.. u e int S,
implies II = 0, and hence D sins = 0. 0
29.12. Locally Regularly Monotone Operators,
Minima, and Stability
We want to study the local minimum problem
1:(11) - b(u) = min!, " eX,
together with the Euler equation
(66)
F'(II) = b,
II e X.
(67)
Let u be a solution of (67). Our goal is to prove important criteria which

680
29. Noncocrcivc Equations and Nonlinear Fredholm ..\Itemati..'es
guarantee that u is a strict local minimal point of (66). In this connection, we
will use locall) strongl}: nwnotone operators and locally regular/}' monotone
operators. We assume:
(HI) The functional F: U(u) e X..... R is defined on a neighborhood U(u) of
the point II in the real B-space X.
(H2) The functional b: X -+ R is linear and continuous.
Recall that u is called a local minimal point of (66) iff
F(v) - b(v) > F(u) - b(u)
for all (.' in some neighborhood of u. Moreover, u is called a strict local minimal
point of (66) iff
f"(v) - b(v) > F(r4) - b(u)
for all v in some neighborhood of fI with v =1= fl.
In nonlinear elasticity, problem (66) allows the following physical inter-
pretation:
II = displacement of the elastic body,
F = elastic potcntial energy,
b = outer force. (68)
b(u) = work of thc outcr force corresponding to the displacement u,
£po,(u) = F(u) - b(u) (potential energy).
Then problem (66) corresponds to the principle of minimal potential energy.
This will be studied in Part IV.
Definition 29.24. Assume (H 1), (H2). Set Epoc = F - b.
(i) Thc point u is called stable with respect to Epo( iff II is a strict local minimal
point of E pOl -
(ii) The point u is called ,,'eakly stable iff the second variation satisfies thc
following condition:
<5 2 F(u; h)  0 for all hEX.
(iii) The point u is called unstable iff 14 is not weakly stablc, i.e., there is an IJ
such that 2 F(u; h) < o.
(i\') The point u is called critically stable iff u is weakly stable and there is an
h  0 such that b 2 F(u; h) = O.
(v) The boundar}' of stabilit}7 consists of the boundary of the set of all stable
points.
Bclo\v, we shall show the following:
(a) If u is a local minimal point of Epo't then u is weakly stable pro\rided <5 2 F
exists at u.

29.12 Locally Rcgularly Monotone Opcrato tinima. and Stability
681
(b) Let dim X < oc. Then u is stable if
(F) c5F(14: II) = 0 and <5 2 F(u: h) > 0 for all II e X - {O}.
(c) Let dim X = 00. Then u is stable if condition (F) holds. and the second
variation c5 2 F is regular at u.
I n Section 18.7 \ve mentioned the classical error of Legendre (1786) in the
calculus of ,'ariations. In modern language, Legendre's error was to think that
(b) above also holds for intinite-dimensional B-spaces X. The reason for this
failure will be explained in Remark 29.30 below.
Let F be C 2 in a neighborhood of u. Then, in terms of locally monotone
operators. we shall sho\v the follo\\'ing.
(a) The point u is weakly stable iff u is locally monotone at u.
(b) Let dim X < . Then u is stable if
E(I') = F(u) - b = 0
and F' is locally stricti}' monotone at u.
(c) Let dim X = 00. Then u is stable if E(14) = 0 and f1' is locally regular/}'
monotone at u.
The notion of regular second variations and locally regularly monotone
operators is closely related to the Garding inequality (cr. Definition 29.38
0010\\' ).
In Part IV we shall discover the following remarkable fact:
Tile elastic potential ellerg>' F of all ela.tic bod}' ;s not a conl..'ex fi"lctional
of the displace"aellt u ill realistic models in elastic;t}'.
This reflects the possible appearance of rupture and plasticity. Thus, the
theory of monotone operators is nOl applicable to realistic models in elasticity,
but only to approximation models. However, locally strictly monotone (morc
precisely, locally regularly monotone) operators are applicable, as we \\'ill
discuss in Section 29.19.
29.12a. Basic Ideas
In order to explain the basic ideas for equations (66) and (67) above, we first
consider the simple finite-dimensional case. The following assertions are
special cases of our results below for arbitrary B-spaces. Recall that the
original problem (66) is identical to the minimum problem
(M) F(u) - b(u) = min!, u e X,
and (61) is identical to the Euler equation
(E)
F'(u) = b
UE x.

682
29. Noncoercive Equations and Nonlinear Fredholm Alternativcs
Proposition 29.25 (Finite-Dimensional Minimum Problems). Assume (H 1) and
(H2) ,,'itl, dim X < 00 (e.g.., X = R h '). Let F be CI: OIl a neighborhood of u with
k > 1. Then:
(A) Necessary condition for a local minimum. If u is a local minimal point of
the origilJal problem (66 then:
(i) F'(u) = b, i.e., II is a solution of the Euler equation.
(ii) F' is locally monotone at u in the case where k > 2, i.e.,
(F"(u)h, h)  0
for all heX.
(B) Sufficient condition for a strict local minimum. Let F(II) = b. Then II is a
strict local minilnal point of (66) if one of lile follo,,'ing ''''0 conditions is
satisfied:
(i) F' is strictl)t monotone on a neighborhood of u.
(ii) F' is locally strictly "Jonotone at u in the case "there k  2, i.e.,
(f....(u)h, h) > 0
ror all II e X - {OJ.
(69)
(C) Sufficient condition for a local minimum. Let F(u) = b. Then u is a local
m;n;,nal point of (66) if one of the follo"tilJg t,vo conditions is satisJied:
(i) F' is nlOIJotone on a IJeighborhood of u.
(ii) F' ;s locally monotone on a neighborhood V of II in case k  2, i.e.,
(FN(v)h, h)  0
for all v e V, hEX.
(70)
From (i) or (ii) it follows that F is ,'onvex on a neighborhood of u.
(D) Sufficient condition for a unique global minimal point. Let fe.: X  X.
be C' ,,,ith k > 1, and let F(u) = b. Then II is tile unique global Inillillial
point of (66) if one of the follo,ving t,vo conditions is satisfied:
(i) F is strictly Inonotone on X.
(ii) f" ;s locally stricti)' monotone on X in case k  2, i.e.,
(F"(v)lt,h) > 0
for all v e X, hEX - {O}.
(71)
Froln (i) or (ii) ;t follo"'5 tllat f" is stricti}' convex on X.
(E) Sufficient condition for a global minimum. Let F: X .-. X. be  \,,;th
k > 1, and let F'(u) = b. Tllen u is a global minimal POillt of (66) if one of
the follo\"ing ''''0 conditions is satisfied:
(i) F' is monotone on X.
(ii) F' is locall) ",onotone 011 X in case k > 2, i.e.,
(F"(v)lJ, h)  0
for all v, hEX.
FronJ (i) or (ii) it follo,,'s that F ;s cOllvex on X.
(F) Eigen\'alue criterion and stability. Let k  2. Along with the Euler equation
F'(u) = b,
UE X,
,,'e ('onsider tile linearized eigellvalue equation of Jacobi:
F"(u)h = ph.
hEX, peR.
(72)

29.12 Locall)' Regularl)' Monotone Operators, Minima. and Stability
683
Suppose that u is a soilltion of the EI4ler eql4ation. Let Jlmin denote the
snwllest eigenvalue of (72), i.e.,
. 6 2 F(u; h)
JJmin = mln Ih1 2'
.. X-(O}
,,'lIere t5 1 F(u; h) = <F"(u)h, h), and IIII denotes the Euclidean norm of h.
Theil:
(i) If Jlmin > 0, then u is a strict local Ininilnal point of ti,e original
minimum problenl (66), i.e., 14 is stable.
(ii) If Pmln < 0, then u is not a local minimal point of (66), and u is unstable.
(iii) Tile point is weakly stable iff Prain  o.
(iv) The point 14 is critically stable iff /Jmjn = O. In this case, the behavior
of Epot = F - b depends sensitit'el}' on the structure of tile higher-
order derivatiL'e.'i f'C')(u) with r > 2.
Moreover, tile Ininimal value of the so-called accessor)' quadratic mini-
mum proble",
t5 2 F(u; h) = min!,
Ihl = 1, heX,
is equal '0 /.lmln.
(G) Local diffeomorphism. Let k  2. The operator F' is a local C-difJeo-
morphisnl (It the point u iff
FN(u)h = 0
implies
II = 0,
i.e., p = 0 is not an eigenvalue of the Jacobi equation (72).
(H) Bifurcation and the Jacobi equation. We no\v consider the problem
F(u,).) - b(u) = min!,
II e X,
(73)
",II ere F depends on the real paralneter A. Tile corresponding Euler eql4a-
tion! reads as follows:
F.,(u, ).) = b,
u e X, l e R, be X.
(74)
Let F: U(U Ot Ao)  X x R --. R be C. on a neighborhood of tile point
(uo, ;"0) with k   and suppose that (uo, lo, b o ) is a solution of (74). The
correspondi1Jg Jacobi equation is given by
F...,(uo, Ao)h = P
heX, It E IR.
(75)
Then:
(i) If /J = 0 is not an eigenvalue of the Jacobi equation (75) (e.g.,
2 F(u, Ao; h) :I: 0 for all h :I: 0) , then the Euler equation (74) Iws
a C- 1 -solution u = u(A,b) through the point (uo,,to,b o ), which is
unique in a sufficientl}' small neighbor/load of tllis point (Fig. 29.9(a».
(ii) If (uo. Ao b o ) is a bifurcation point of the Ellier equation (74) ,vith
respect to the parameter (A, b), tl,en p, = 0 is an eigenvalue of tile Jacobi
I In Section 29.13 \'I"e consider the buckling of beams. In this case. tbe parameter .( corresponds
to the outcr boundary force.

684
29. Noncoera\'c Equations and Nonlinear Fredholm Allernati..'es
u 1
II t
(uo' Ao. b(l)
. (A. b)
.-. (A. b)
(a)
(b)
Figure 29.9
equation (75), i.e., there is an h:l: 0 such that (j2 F(uo, ;'0; h) = 0
(Fig. 29.9(b).
(iii) COIJversel}'. if F has the stnlclure
F(u, A) = (A - o)a(u - 1'0' U - uo) + r(lI, i.),
then (14 0 , 4) is a bifurcatiolJ poilJt of the ElIler equatioll (74) ,,'itl. b = 0
ill tile ca!e ,,'''ere a: X x X  R ;s a bilinear, S}'II.metr;c, str;cll) posi-
tive fUllctional. alul
I . IIr(u. ,t)1I 0
1m 2 = ,
., "'''0 1111 - 1'0 II
rlnifoYlnly for all A in a suffic;elJtl}' small IJeighbor"ood of Ao.
This proposition shows clearly the importance of the theory of monotone
operators and of spectral theory for minimum problems and stability theory.
The crucial connection between stability and bifurcation contained in Pro-
position 29.25(H) will be discussed in Remark 29.29 below. Assertions (i) and
(ii) in (H) above Collow from the implicit function theorem (Theorem 4.8) and
Proposition 8.2, respectively. The proof of (iii) in (H) will be discussed in
Problem 29.7. This proof is extremely simple in the case where the remainder
r( · ) docs not depend on the parameter i...
EXAMPLE 29.26. Let X = R, and let F: U(u) C X -tt R be C 2 on a neighborhood
of u. Then
(F'(u)h, h) = f:N(u)h 2
and hence we have that:
(i) F' is locally strictly monotone at u iff F"(u) > O.
(ii) F' is locally monotone at u iff FN(u)  O.
(iii) F' is (strictly) monotone on the open interval U iff F' is (strictly) mono-
tonically increasing on U.
Consequently, Proposition 29.25 and the following results below generalize
well-known properties of real functions. Figure 29.10 corresponds to the case
b = O.
for all h e iii,

29.12. Loeall). Regularly Monotone Operators. 4inima.. and Stabilit).
685
.
-F
V-F
..
--.- - t
U
.
It
(a) [.()Cal minimum
(F'(u) = 0 and Fry(lt) > 0)
(b) Strict local nlinimum
(F'(lI) = 0 and F"(u) > 0)
I
I
F
t
II
(c) Global nlinimunl
(F N {,,) > 0 for all ,.)
..
Figure 29.10
EXAPttPLE 29.27. Let X = (R.Jl, and let F: U(u) s; X -+ [R be C 2 on a neighbor-
hood of u. Then
ftt'
F'(II)h = L D;f"(u)h"
j=1
N
(F"(u)/.. h) = L D;D j F(u)lI i lJ j
'.J= I
where h = (11 1 ,...,11".), II = (u.,...,UN)' b = (b 1 ,...,b...), and D i = O/OUi' The
Euler equation F'(u) = b is equivalent to the system
for all hEX,
D.F ( u ) = b.
I I'
i= 1,...,N.
The Jacobi equation F"(u)h = plJ corresponds to the eigenvalue problem
N
L DjDjF(u)lJ j = ph;,
)=1
i.e., the eigenvalues JJ l' 1l2' ... of the Jacobi equation are identical to the
eigenvalues of the symmetric matrix (D,D J F(u»"j=l.....".. Then:
(i) f.' is locally strictly monotone at II iff Pi > 0 for all i.
(ii) F'(u) is locally monotone at II iff P,  0 for all i.
; = I, "" N,
Remark 19.28 (The Importance of Jacobi's Eigenvalue Criterion). It is quite
remarkable that the eigenvalue criterion in Proposition 29.25(F) can be
generalized to large classes of problems in infinite-dimensional B-spaces and

686
29. Noncoerci,'c Equations and Nonlinear Fredholm Alternatives
(V)
hence it can be generalized to variational problems. This will be ShO\\'D below.
In order to explain the basic idea of this fundamental technique let us considcr
the variational problem
fG !(ul + u) + (j(u) - bu)dx = min!,
u = 0 on oG,
where G is a bounded region in fR2. If u is a solution of (V), then u satisfies the
Euler equation
(E)
-u + I'(u) = b on G,
u = 0 on iJG,
which corresponds to F'(u) = b. The linearized Euler equation F"(u)1J = b is
obtained from the Euler equation by replacing u with u + th and differentiat-
ing with respect to t at t = O. This yiclds
-l1h + fN(U)h = b on G,
h = 0 on oG.
Consequently, the Jacobi equation F"(u)1a = ph corresponds to
(E un )
- h + 1"(u)1I = ph on G.
h = 0 on oG.
If the smallest eigenvalue Pmin of(J) is positive, then we expect that the function
r4 corresponds to a strict local minimum of the original problem (V), i.e., u is
stable. It follows from our general results in Section 29.19 that this criterion
is true in the case where the functions u and f are sufficiently smooth.
(J)
Remark 29.29 (Loss of Stability and Bifurcation). We want to show that
Proposition 29.25 (H) represents the simplest model for the following two
important principles in physics.
(PI) Bifurcation cannot occur at stable states.
(P2) Loss of stability of a state with respect to an outcr parameter can lead
to bifurcation.
To explain this, we will use the language of elasticity introduced in (68)
above. Principle (PI) is an immediate consequence of Proposition 29.2S(H).
We now discuss (P2). In Proposition 29.2S(H) (ii), the clastic potential
energy has the form
F(u, ).) = (;. - o)a(u - u o , u - uo) + o( lIu - "011 2 ), U -. uo,
where A is an outer parameter. Since the symmetric bilinear functional a(., .)
is strictly positive, we have:
Uo is stable if l > Ao;
Uo is unstable if A. < lo.

29.12. Locally Regularly Monotone Operators. Minima. and Stability
687
u
U2
Uo
stable
/
boundary of
stability
liS
unstable
critically stable
-'A
b,
Ao
(a)
(b)
Figure 29.11
Roughly speaking, this change of stability of Uo is responsible for the bifurca-
tion of the Euler equation fI(u, ).) = 0 at (uo, lo)' To understand this, we
consider the simplest possible case, namely,
f(u, l) = l(A - Ao)(U - u o )2,
where u and l arc real numbers. In fact, the Euler equation
Fy(u, l) = (l - 4)(u - uo) = 0
has the bifurcation point (u o , Ao) (cf. Fig. 29.11 (a»).
As a second example for (P2), we consider the minimum problem
F(u) - b(u) = min!, u e R 2 ,
with F(u) = U1U + ufj3 and b(u) = blu, + b2U2t where we regard b as an
outer parameter. The Euler equation F(u) = b has the form
(E)
u + u = b l ,
2u. U2 = b 2 ,
and the Jacobi equation FN(u)h = ph reads as follows:
(1) ( 2U 1 2U2 )( ht ) = P ( hl ) .
2U2 2uI h 2 h 2
Obviously,thepointu 1 = 0,112 = O,iscriticall}'stable,sincePrain = 0. Further-
more, this point lies on the boundary of stability. In fact, in each neighborhood
of (0,0), there arc points (u l' U2) with Jlmin > O.
Figure 29.11 (b) shows the solution set of equation (E) and its stability
properties for b 2 = 0 and b l e R. In this case, b l plays the role of a bifurcation
parameter. In fact, the point u = 0, b = 0, is a bifurcation point of the solution
set of (E) \\'ith respect to the parameter b = (b l , 0). For b 2 = 0, the two solution
branches of (E) are given by
u = b J ,
U 1 = 0
and
u = b l ,
Ul = 0.

688
29. Noncoercive Equations and Nonlinear Fredholm Ahernati\'es
In order to avoid misunderstandings, note the following. If the Cunctional
F: D(F) £ X -t R is C 2 on the open set D(F) of the real B-space X. then the
set of all the solutions (u, b) of the corresponding Euler equation F'(II) - b = 0
Corms a CI-manifold M in the product space X x X., according to the
preimage thcorcm (Theorem 4.J). In contrast to this fact, the intersection of
M with a linear subspace of X x X* need not be a manifold. This can lead
to bifurcation with respect to appropriate parameters b living in a subspace
of X*. For example, the set of all the solutions (u. b) of equation (E) abo\'c
forms a c..-x; -manifold JW in R4. The solution set of Figurc 29.11 (b) corresponds
to the intersection between j\f and the hyperplane b l = O. From a physical
point of view, the restriction oC the parameters is meaningful. For example, in
Section 65.7 we shall study the buckling of clamped plates. In this case. the
outer boundary forces b have no vertical component.
Remark 29.30 (Typical Difficulties in the Case where dim X = 00). In the case
where dim X < oc, the local strict monotonicity oC F' at u, i.e..
(FN(u)h, II) > 0
for all hEX - {O}
(76)
is equivalent to the local strong monotonicity of F' at u. i.e.,
(F"(u)lI,h)  cHlln 2 for all heX and fixed c > O. (76*)
In fact" iC we set a(h, k) = (feN (lI)h, k). then the bilinear functional a: X x X -+
R is symmetric. and it follows from (76) that a(., · ) is strictly positive. Since
dim X < 00, thc functional a( ., · ) is also strongly positive.
Observe that this argument fails in the case where dim X = 00. Roughly
speaking, this follows from the fact that the positive eigenvalues of a( ., · ) may
converge to zero. This causes the typical difficulties in the calculus of varia-
tions. We have:
If F'(u) = 0, II e X, and dim X = 00. then the local strict monotonicily of F
alII does 1101 guaralltee tllat F lias a local minimum at II.
We will show below that one has to replace "locally strictly monotone at u"
by "locally strongly monotone at un or "locally regularly monotone at u." The
latter condition plays a Cundamental role in the calculus of variations as we
shall see be I 0\\' .
Remark 29.31 (Importance of Variations). Assume (HI). In Proposition 29.25
we supposed that F is C 2 . However, this assumption is too strong with respect
to many applications in infinite-dimensional B-spaces X. Thereforc. we y,'ork
with variations. Recall the following from Chapter 4. Let
q)(I) = F(u + th)
for all real numbers in some neighborhood of t = 0 and fixed u and " in X.
By definition, the nth variation of f. at the point u in the direction oC IJ is

29.12 Locall)' Regularl)' Monotone Operators. Minima. and Stability
689
given by
b" F(u; h) = tp(ftJ(O),
n= 1,2,...
(77)
We say that the 11th variation b" F exists at the point 14 iff b" J:(u: h) exists for
all heX. If F is Crt in a neighborhood of II, then bllF exists for all v in a
neighborhood of u and
c5" F(t: IJ) = F(It)(V)IJ" for all heX. (78)
For n = 1, 2, this can be written in the form:
£5 F(l': h) = F'(v)IJ,
l F(t,; h) = (F"(v)h, I,)
for all heX.
(79)
In the following. a condition like "b 2 F(II, IJ)  on includes tacitly the existence
of b 2 F(rl; II).
Remark 29.32 (First Reduction Trick). Assume (H I) and (H2). We set
1/1(1) = F(u + lh) - b(u + Ih)
(80)
for all real numbers I in some neighborhood of I = 0 and fixed II and II in X.
Note that b(II + III) = b(u) + tb(/a). Obviously, we have
"'(1) = tSF(u + th: II) - b(IJ),
tJlH(I) = c5 2 F(u + ria; h),
(81 )
in the case y,'here the corresponding variations exist. Using the real [unction
t/J, the proofs belo\\' \\rill follow I,,'er}' easil)7 from the corresponding \\'ell-knoy,'n
properties of real functions.
Remark 29.33 (Second Reduction Trick). Boundary \'alue problems lead
frequently to problems of the following form:
F(u) - b(u) = min!, u E g + X, (82)
where we assume that ".g + X" makes sense, i.e., we assume that there is a
linear space L such that X s Land geL. In elasticity, for example. 9 corre-
sponds to the displacement of the boundary of the elastic body. Suppose that
b: L ..... R is linear. No\\', if we set v = u - g, then problem (82) is equivalent
to the following problem:
G(tt) - b(v) = min!, veX, (82-)
\\'here G(I') = F(g + v). Using this simple method, all the follo\ving results can
also be applied to problem (82). In this connection, note that
"F(u; h) = "G(v; h)
for all hEX,
where II e 9 + X and u = 9 + v.

690
29. Noncocrcivc Equations and Nonlinear Fredholm Altcrnati\'cs
29.12b. Necessary Conditions for Minima via
Locally Monotone Operators
Proposition 29.34. Assume (H I) and (H2) above. Suppose that u ;s a local minimal
point of tIle problem
F(u) - b(u) = min!,
ue x.
(83)
TIJen:
(i) If ,he first variat ion l: exists al fl, the"
tSF(u; II) = b(h) Jor all heX.
(ii) If the second variation 2 F exists at u, then
tS 2 F(u; h) > 0 Jar all heX.
(84)
(85)
Corollary 19.35. Assrlme (H I), (H2), a,ul assume II,at F is C 2 in a neighborhood
of u. Suppose thaI u is a local minimal point of (83). Then
f"(fl) = b,
(FN(u)h,h)  0 for all heX,
i.e., F' is locally monotone al u.
(84*)
(85*)
PROOF. For fixed heX, we set
t/J(t) = F(u + th) - b(u + th).
where t is a real number in some neighborhood of t = o. If u is a local minimal
point of (83), then t = 0 is a local minimal point of the real function t/J. Hence
""(0) = 0 and "'''(0)  o.
This is (84) and (85).
The corollary follows from (79).
o
29. 12c. Sufficient Condition for a Strict Local Minimum via
Locally Strongly Monotone Operators
The key condition reads as follows:
c5 Z F(u; h)  C 111111 2
for all hEX and fixed c > o.
(86)
Proposition 29.36. Assume (HI), (H2), and assume that:
(i) of(u; h) = b(lI) for fixed u and all II EX, a,1d condition (86) holds true.
(ii) The second variation tS 2 F exists on a neighborhood of u, and for each

29.12. Loeall)' Regularl)' Monotone Operators, Minima, and Stability
691
I; > 0, there ;s an ,,(£) > 0 such tl,at
1<5 2 F(v; h) - <5 2 F(u; 11)1 S e 1111 11 2 .
.(or all h, veX \vith nv - ull < ,,(£).
Then 11 is a strict local minimal point of (83).
Corollary 29.37. ASSllme (Hl (H2). Lel F be C 2 in a neighborhood of II.
Suppose that F'(u) = b and that F' is locally strongly monotone at II, i.e.,
(F"(u)h,h)  cllhll 2 for all hEX and fixed c > o.
Then u is a strict local minimal point of (83).
PROOF. For fixed heX, we set
t/I(l) = F(u + th) - b(u + th)t
where the real number' lies in some neighborhood U(O) of t = O. By (ii), the
second derivative "'''(l) exists for all t E U(O). The classical Taylor theorem
tells us that
,2
I/I(t) = ';(0) + 11/1'(0) + 2" ';"(81)
for allt E U(O) and 0 < f) < 1, where 8 depends on t. By (i), ';'(0) = 0 and
t/1"(O) > cUhU 2 .
Let 1 t 1  1. It follows from (ii) that
1';"(91) - 1/1"(0)1 = Ib 2 F(rl + 3th; h) - 2 F(u; h)1 S I: II h 11 2
for all II E X with 11/1 U < ,,(t). Set t = c12. Then, for all hEX with IIh II < '1t we
obtain
1/1(1) > ';(0) + : II h 11 2
for all heX..
(87)
i.c., II is a strict local minimal point of (83).
The corollary follows from (79).
o
If the space X consists of smooth functions, then the key condition (86)
cannot be verified for variational integrals. Our goal is to weaken the condition
(86) in order to obtain a fundamental sufficient criterium for local minima in
the calculus of variations. The simple idea is to show that the inequality (87)
is valid
when IIhlf 2 is replaced by IIhll,
where 11.11, is a weaker norm on X, i.e., Uhlir  constilhll for all hEX. When
applying the following results to variational problems, one uses typically the

692
29. Noncocravc Equations and Nonlinear Fredholm Altemati\'cs
follo\ving spaces:
X = {u E C'"( G ): lY'u = 0 on aG for lal  n. - I}.
Y = W 2 "'(G), Z = L2(G)
where G is a bounded region in R"., N  1, and m  1.
29.12d. Locally Regularly Monotone Operators
The ke)p condition is the follo\\'ing Garding inequality:
a (h. II) > (II h II  - C II h II 
for alii! e Y with fixed c > 0 and C > 0, where
(88)
2 f"(u; h) = a(h, Ia)
for all I. E X.
(89)
and X s Y s Z, i.e., a(., .) is an extension of the second variation from X
to the larger space Y.
Definition 29.38. Let F: U(u)  X --. R be a functional on a neighborhood of
the point u in the real B-space X. The second variation 2 F is called regular
at the point rl iff the follo\ving conditions are satisfied.
(i) b 2 F exists on a neighborhood of u.
(ii) There exist real H-spaccs Y and Z such that the embcddings X s; Y c Z
are continuous and the embedding Y c Z is compllcr.
(iii) There exists a bilinear, symmetric, bounded functional a: Y x Y -+ R such
that (88) and (89) hold, i.e., a(., .) is a regular Gdrdi'19 jornJ.
(iv) The second variation is uniforml.v continuous with respect to the larger
space Y, i.e., for each £ > 0, there is an ,,(t) > 0 such that
12 F(v; h) - tS 2 F(u: h)1 < I: II h II.
for all v, II e X with I! v - ull x < " (l:).
If condition (88) holds with C = O. then the space Z drops out.
This concept corresponds to the general strategy in analysis of using set'eral
spaces in order to obtain sophisticated results.
Definition 29.39. Let F: U(u) e X..... IR be C2 on a neighborhood of the
point u in the real B-space X. Then, F' is called locally reglllarl}' monotone at
the point u iff F' is locally strictly monotone at u. and the second variation
2 F is regular at II.
Recall that 2 F(v; h) = (F"(v)lJ, h).

29.12 Locally Rcgularly Monotone Operators. Minim and Stabilit)'
693
29.12e. Sufficient Condition for a Strict Local Minimum via
Locally Regularly Monotone Operators
The ke}' condition reads as follows:
c5F(u: h) = b(h)
for all heX.
a(lI II) > 0 for all heY - {O},
\vhere c5 2 F(u; II) = a(h, II) for all hEX and X c Y c Z. We assume:
(H) Let F: V(II)  X -+ R be a functional on a neighborhood of the point"
in the real B-space X, and let b E X. be given. Suppose that the second
variation <5 2 F is regular at u in the sense of Definition 29.38 abo\'e. Let
Y #= {O}.
(90)
Theorem 29.H. ASSfllne (H) and assfll1le that cOllditioIJ (90) is sati(ied. TheIl"
is a strict local 111illilnal point of the problem
F(II) - b(u) = min!,
ue X.
(91)
MoreOl,er, there are numbers d > 0 and e > 0 such tllar
Epoc(v) - Epoc(u) > dll v - 111I (92)
for all v E X \\-'ith IIv - ull r < t. \'here \\-'e set Epot(v) = f'(v) - b(v).
Corollary 29.40. Let F: U(u) e X... R be C 2 on a neighborhood of the poi"t
u in tile real B..pace X, a"d let b e X. be given. SllppOse tllat
1:'(u) = b,
alld .uppose tllat F' is locall)7 regularly monotone at tile point II. TheIl U is a
strict local l1Ji";l1Jal point of (91).
In terms of elasticity. condition (92) says that small changes of the potential
energy Epot correspond to small changes of the displacement II with respect to
the norm n .11 y. Thus, inequality (92) describes the stabilit}' of the state" of the
elastic body. The norm 11.11 r is called the energetic norm.
The following proof is essentially based on the Hestenes theorem (Proposi-
tion 22.39).
PROOF. It follows from (90) tha't a: Y x Y  R is strictly positive. By Proposi-
tion 22.39, the GArding form a(., .) is strongl}' positive. i.e., there is ad> 0
such that
a(lI, h) > d nhll for all heY.
We set t/J(t) = F(u + lh) - b(u + tll) for fixed II e X and real t in a neighbor-

694
29. Noncocrcive Equations and Nonlinear Fredholm Alternatives
hood or t = O. As in the proof or Proposition 29.36, we obtain that
c
1/1(1) > 1/1(0) + 4"hl.
(93)
for all heX with 1!I'lI r < '1. Since the embedding X c Y is continuous we
have Uhlir S const 1I'llix for all heX. Thus, there is an '10 > 0 such that (93)
holds for all heX with IIhllx < '10. 0
The corollary is an immediate consequence of Theorem 29.H by Definition
29.39.
29. 12f. Sufficient Condition for a Strict Local Minimum via
Linear Eigenvalue Problems
Along with the original minimum problem
(P)
F(u) - b(u) = min!,
u EX,
we consider the so-called accessory quadratic n,inimum problel11
(A)
a(h,h) = min!
(hlh)z = 1
and the eigent'il/lle problenJ of Jacobi
heY,
(E) a(lJ, k) = Jl(hlk)z,
for fixed II E Y - {OJ, peR, and all k E 1':
Recall that a(la. h) = t5 2 F(u; h) for all heX. Moreover, the embeddings
X c Y c Z arc continuous, and the embedding Y  Z of the H-spaces Yand
Z is cOl1apact.
Theorem 29.1. Assume (H) frona Theorem 29.H, and assume that
t5 F(u; II) = b(h) for all heX.
Then u is a strict local nJin;mal point of the original problem (P) in the ,.ase
where one of the follo,,-ing four mutuall}' equit'illent conditions is satisfied:
(i) Strict Legendre condition: a(h, h) > 0 for all heY - {OJ.
(ii) Strong Legendre condition: a(ht II)  d II h II  for all heY and fixed d > O.
(iii) Accessory problem. The infimum of problem (A) is positive.
(iv) Eigenvalue criterion: The smallest eigenvalue p of the Jacobi equation (E)
is positive.
CoroUary 29.41. Assume (H). Then the accessory problem (A) lIDs a solution,
and the nlinimal value Ilmin of (A) ;s tIle smallest eigenvalue of (E). Let

29.12. Locally Regularly Monotone Operators. Minima. and Stabitit),
695
c5F(u; h) = b(lJ) for all II EX. 1.hen:
(a) If l'mlD > 0, the,. u ;s a strict local minimal poi,.t of(P} and u is stable.
(b) If Jlmin < 0 and X is dense ;n Y, then u is not a local mi"imal point of (P)
(lnd 14 ;s unsl able.
PROOF OF THEOREM 29.1. (i) <:>(ii). This follows from the proof of Theorem 29.H.
(ii) => (iii). Since the embedding Y s Z is continuous, we have IIhU z 
const IIhll r for alii. E 
(iii)  (i). This is ob\'ious.
(iii)<:> (iv). This follows from Theorem 22.G. Notc that, in the case of the
smallest eigenvalue, the proof of Theorem 22.G does not depend on the
separability of the H-space.
If (i) holds, then u is a strict local minimal point of (P), by Theorem 29.H.
o
PROOF OF COROLLARY 29.41. The first statement follows from Theorem 22.G.
Ad(a). This is a consequence of Theorem 29.1.
Ad(b). If J.lmln < 0, then there exists an I. E Y such that a(/., h) < O. Since
a: Y x Y -. R is continuous and X is dense in  there is an h EX such that
a( h , h) < O. and hence b 2 F(I4; " ) < O. 0
Theorem 29.1 is an abstract variant of the classical theory of Jacobi men-
tioned in Section 18.7.
We now want to study the behavior of the Euler equation
F'(u) = b,
ue X,
(94)
in a neighborhood of a fixed solution u. To this end, we will use the strict
Legendre condition in the weak form
t5 2 F(u;h) > 0 for all hEX - {O}. (94-)
Proposition 29.42. Let F: U(u) c X -. iii be Cl: on an open ,aeigllborhood of the
point u i'l the real B-space X, ,vhere 2  k S co. Let u be a solut;on of the Euler
equalio'l (94). Suppose that (94*) holds and that FN(u): X -. X. is Fredholm of
index zero.
Then the operator f: U(I4)  X  X. is a local C"-diffeomorphism at Ilae
po;"t u.
This proposition tells us the following important fact. There are neighbor-
hoods V and W of II and b, respectively, such that the equation
F(ii) = b, U E 
- -
has a unique solution Ii for each givcn b e Jt: Morcover, if we set II = G(b),
then the solution operator G is C" on  Figure 29.12 illustrates the intuitive
meaning of this result.

696
29. Noncocrcive Equations and Nonlinear Fredholm Alternati..-es
.
F'
b
.
II
u
Figure 29.12
PROOF. If F N (II)h = 0, then (l'-"(u)h. h) = 0 and hence h = 0, by (94-). Thus,
the assertion follows from Proposition 29.17(i). 0
29.12g. Sufficient Condition for a Global Minimum via
Convexity
We \\'ant to stud)' the minimum problem
F(II) - b(lI) = min!,
u e C,
(95)
\\'here F is convex on the convex set C (e.g., C = X). Let b e X*.
Proposition 29.43. Let F: C !;; X -+ R be convex on Ille convex subset C of
the real B-space X. Then each local nlilJinlal point of (95) ;s also a global
mi/lillwl point.
If, in {Idditioll, F is stricti)' convex on C, tl,en the global ",inimal poi"t u ;s
unique
PROOF. Set G(v) = J:(v) - b(v). Let u be a local minimal point of (95) and
suppose that there is a v E C such that G(v) < G(u). Since G is convex, we
obtain
G«I - l)U + tv)  (1 - t)G(u) + tG(v) < G(II)
for all t e ]0, I].
For small t, 'Are obtain that u is not a local minimal point. This is a contradic-
tion. The uniqueness of the minimal point follows from Corollary 25.15. 0
Proposition 29.44. Let F: C  X -+ R be a functional on the open convex
neighborhood C of t/Je point fl in the real B-space X. Let
F(II; 11) = 0 for all IJ e X.
Suppose that b 2 F exists at eCle/J point of tIle set C and suppose that
c5 2 F(v; h)  0 (resp. b 2 F(v; IJ) > 0)
.for all t' e C and all heX (resp. all heX - {O}).
Then II ;s a global I,Jininlal point of(95) (resp. fl ;s the unique global minimal
point of (95». Moreover, F is cont"ex (resp. strictly convex) OIl C.

29.13. Application to the Buckling of Beams
697
Recall that
c5 2 F(v; h) = <F"(v)ll h)
in the case where F is C2 on the set C.
forall veC, heX.
Corollary 29.45. Let F: C £; X -+ R be C J on ti,e ope" CO'IL'eX neighborhood C
0.( the point u in Ihe real B-space X. Let F'(u) = b. Suppose that F is nlolJolo'Je
(resp. stricti)' monotone) on C.
Then tile asserlions of PropositiolJ 29.44 hold true.
PROOF. We set t/J(t) = G(u + th), where G = F - b. and  u + h e C. For all
1 E [0, I],
t/J'(t) = c5F(u + Ih; II) - b(h), '" "(I) = 6 2 F(u + Ih; h).
If F is C. on the set C. then ""(I) = (F'(u + th) - b,h).
The functional G is (strictly) convex on C iff it is (strictly) convex on each
line in C, i.e., the real function'" is (strictly) convex on [0, 1] for each hEX
with u + " e C.
Now use the following \\'ell-known property of real functions. It follows
from either
t/JH(t) > 0 (resp. ""'(1) > 0)
for all I e [0, I],
(96)
or
t/J' is monotone (resp. strictly monotone) on [0, 1], (96*)
that t/I is convex (resp. strictly convex) on [0, 1].
Finally, note that the assumptions of Proposition 29.44 and Corollary 29.45
ensure (96) and (96*), respectively. 0
29.13. Application to the Buckling of Beams
We investigate the buckling of a clamped beam of length I under the influence
of the boundary force P > 0 as pictured in Figure 29.13. According to Euler,
we use the following classical variational problem:
Epot(u)  f (!Acp'z + P(cos cp - I»dt = min!,
q>(0) = lp(/) = 0,
which corresponds to the principle of minimal potential energy. The cor-
responding Euler equation is given by
(97)
H P. 0
tp + A sin  = ,
q>(0) = <1'(/) = o.
(98)

698
29. Noncocrci\'c Equations and Nonlinear Fredholm Alternatives
f

t
p
beam
/
T. p
· t
o
(a) P < P CN
(b) P> p,"
Figure 29.13
A detailed physical motivation for (97) will be given in Section 64.7 of Part
IV. Here, the parameter t denotes arclength counted from the origin 0, and
({J denotes the angle between the tangent at a point of the beam and the -axis.
Note that tp' corresponds to the curvature of the beam. The positive parameter
A depends on the material of the beam. The precise meaning of A will be
discussed in Section 64.7. Set
11<1'11 = max 1q>(T)I.
OSt1
From the physical point of vie\\', we expect a behavior of the beam as
pictured in Figure 29.13:
(i) If the force is small enough, i.e. P < Pcri" then there is no buckling.
(ii) If the force becomes supercritical, i.e. P > Peril' then buckling occurs.
The decisive problem of Euler was to calculate Peri'. From Proposition 29.48
below we will obtain for the first buckling that:
P = Pcrit(t + s2 + O(S3»
1t 2 A
Peril = 1 2 .
qJ('r) = s sin ; T + 0(5 3 ),
where s is a small real parameter. This solution corresponds to Figure 29.13(b).
Mathematically, we have the following situation. The Euler equation has
the trivial solution cp == 0 for all P. However, this solution looses its stability
at Peril' and at this point, a new stable solution appears (Fig. 29.14(a». To
understand the bifurcation mechanism, we first study the following simple
model in AI:
S -+ 0,
F(u) dcr 1 U2 + P(cos u - t) = min!
u e AI,
(99)
where P > o.
EXAMPLE 29.46. The Euler equation to (99) is given by
F(u) = u - P sin u = 0

29.13. Application to the Buckling of Beams
699
119'11
u
stable -
I
p
stable
I
Pcnt
I
unstable
L-.p
(a)
(b)
Figure 29.14
with the following two solutions:
(i) II = O. P = arbitrary.
2
(ii) P = P(u) = I +  + 0(14 3 ). 14 -+ O.
In addition, we have:
FN(O) = 1 - P for (i),
u 2
F"(P(u)) = 3" + 0(14 3 ). 14 - 0 for (ii).
Thus, the trivial solution r4 = 0 is a strict local minimal point of (99) for P < I,
and a strict local maximal point for P > 1. Figure 29.14(b) shows the solutions
of (99) in a neighborhood of the line rl = 0 and their stability.
This analogy is remarkable. Already, in Chapter 7, we have encountered
the important fact that the behavior of the solutions of semilincar elliptic
partial differential equations can be understood by considering simple
analogous models in 1li 1 .
Remark (Morc General Models for The Beam). Problem (97) corresponds to
the simplest nonlinear model for a beam. The general form of the solutions of
the Euler equation cp" + P A -1 sin cp = 0, independent of boundary conditions,
will be considered in Proposition 29.49 by means of eUiptic functions. The
boundary condition cp(O) = tp(/) = 0 in (98) postulates that the beam is hori-
zontal at the two end points.
If we represent the shape of the beam by the curve
, = a + a(a), , = c(a), 0 S  S 
where , , are Cartesian coordinates (Fig. 29.13) and u = (a, c) is the dis-
placement vector, then it is possible to formulatc more general variational
problems than (97) and to consider more general boundary conditions (e.g..
a(O) = c(O) :: 0 and a(l) = 0). This will be studied in Problem 29.12.
In what follows we will investigate problem (97) in detail.

700
29. Noncoerci\'e Equations and Nonlinear Fredholm Alternatives
29.13a. Heuristic Arguments
In order to explain the basic idea as clearly as possible, we start with heuristic
considerations based on Remark 29.28 above. Let qJ = cp(t) be a solution of
the Euler equation (98). As in Remark 29.28, linearization of (98) at cp yields
the Jacobi equation:
-II N -  hcoscp = ph,
h(O) = h(l) = O.
The sign in (100) has been chosen in such a way that the eigenvalues Jl of (1 (0)
are bounded bclo\v. Let J.lmin be the smallest eigen\'alue of (100). Similarly, as
in the finite-dimensional case. we expect the following:
(i) If Jlmin > 0, then cp is a strict local minimal point of the variational problem
(97), i.e.. cp is slable.
(ii) if Jlmln < 0, then cp is 1101 a strict local minimal point of (97), i.e., cp is
unstable.
( 1(0)
Case I: Let cp = O. Then equation (I (0) has the eigensolutions
h · nn
(T) = slOTT.
n 2 n 2 P
J.l = 12 A ·
n = I, 2, .."
and hence
n 2 p
J.lmin = r - A '
This yields the famous formula of Euler:
1[2A
Peril = 1 2 .
i.e., the trivial solution cp = 0 is stable for P < Peril and unstable for P > Perla.
Case 2: If tp  0 is a solution of the Euler equation (98). then we have to
check the sign of JAmin'
In Chapter 8 we studied in detail the bifurcation at simple eigenvalues. In
the following, we want to show that our general functional analytic results
from Chapter 8 allow us to construct very easily solutions bifurcating at
P = P': rll and to investigate their stability. Roughly speaking. it follows from
Section 8.17 that the bifurcating solutions are stable in the case where the
bifurcation is supercritical, as pictured in Figure 29.14, and as expected for
the beam.
29.13b. A General Result
In order to see that our method does not depend on the special form of the
variational problem (97), we consider the more general problem

29.13. Application (0 the Buckling of Beams
701
F(q» dcf f !q>'2 - Pf(q»dt = min!,
(,0(0) =  tp(l) = Pt
where  fJ, P, and I are fixed real numbers. We also consider the corresponding
Euler equation
(101)
- cpN - Pf'(qJ) = 0,
(,0(0) =  tp(/) = Pt
( 102)
and the Jacobi equation
_IJH - PfH(tp)h = ph, Jl E 
(,0(0) = q>(/) = O.
Here t P and Jl are real numbers. Let Jlmin denote the smallest eigenvalue of
(103). Let p e C J [0, I] with p(O) = oc, p(/) = p. We need the following spaces:
X = {<p E C 1 [O./]: (,0(0) = tp(l) = O},
Y = W 2 1 (0, I Z = L 2 (0, I)
as well as V = C 2 [0, I]" X. Then problem (101) can be written in the
following form:
( 103)
f(q» = min!.
tpEP+X.
(101.)
Proposition 29.47. Let f. R -+ R be C 2 , and let q> be a C 2 .solution of tile EIlier
eqllat;on (102). Then:
(a) If 1Jmin > 0, thell cp is a strict local minimal point of (101.), and (,0 ;S
stable.
(b) If Jlmia < 0, then (,0 ;s not a nJinimal point of (101*), and cp is unstable.
PROOF. According to Remark 29.33, it is sufficient to consider the homo-
geneous case with (X = (J = 0, i.e., p = o.
(I) We show that the first variation vanishes. For II e X, we set "'(I) =
fe(q> + th). Then
""(0) = bF(q>; h) = f h' q>' - Pf'(q»h dt,
1/1"(0) = 2 F(q>; h) = f h'2 - Pf"(q»h 2 dt.
For all hEX, it follows from (102) that
bF(q>;/l) = f (-q>" - Pf'(q»)hdt = O.
(II) We show that the second variation is regular at tp. By the inequality of

702
29. Noncoerciye Equations and Nonlinear Fredholm Alternatives
Poincare- Friedrichs, there is a c > 0 such that
f h,2 df > C f (h'2 + ,,2)df for all hEY.
We set
a(h,g) = f h'g' - Pf"(q»hgdt.
Hence
<5 2 F(q>; h) = a(h, h)
Moreover, for all h e 
a(/J, h) > c IIhll - const  hili.
Thus, b 2 F is regular at tp.
(III) The eigenvalue problem
for all hEX.
a(h.g) = Jl(hlg)z for fixed hEY and all 9 e Y (104)
is obtained from the Jacobi equation (103) by using integration by parts.
Hence, the generalized problem corresponding to (103) is identical to
(104). According to the regularity theory for linear strongly elliptic
equations, each solution of(I04) is a classical solution of(103) and vice
versa. Thus. the smallest eigenvalue of (104) is identical to /Jmin.
Now, the assertions follow from Corollary 29.41. 0
Proposition 29.48 (Bifurcation). Let f: IR --. R be analytic with f'(O) = 0 and
/"(0) > O. Let  = fJ = O. We set P erit = 1t 2 /12f"(0). The'J:
(a) The poi,., (tp, P) = (0, P erit ) is a bifurcatio'J point of the Euler equation (102)
in the space V x R.
(b) In a sufficiently small neighborhood of (0, Perie) ill X X R, there exists a
unique curve through the poi'Jt (0, Pc:ri.) which consists of ,wntrivial solutions
(cp, P) of the Euler equation (102). This curve depends analyticall)1 on a
small real parameter s.
(c) Let /"'(0) = o. Then the soilltions have the following form:
(f'(t) = ssin 7 t + 0(S3). S - 0,
P = Perle + t2 S2 + 0(S3),
Pmin(S) = 2f"(0)£2s2 + 0(S3),
,,'here I: 2 = - f(41{0)1[2 /8/ 2 f" (0)2 .
(d) The trivial solution cp = 0 ;s stable for P < Peril and unstable for P > Prit.
(e) The nontrivial solution if) in (c) is stable for £2 > 0 (supercritical bifurcation)
a"d unstable for £2 < 0 (subcritical bifurcation).

29.13. Application to the Buckling of Beams
703
In the case of the beam, we have f(tp) = A-1(1 - costp), and hence £2 > O.
PROOF. We \\'ill apply Theorems 8.A and 8.E. The following proof proceeds
completely analogously to Example 8.31. We set
P = Peril + t.
Let
v = {cp e C 2 [O, I]: tp(O) = cp(/) = O},
w = C[O, I].
(I) Operator equation. We write the Euler equatioll (102) in the form
H(tI',t) = 0, tp e V, £ e R. (105)
The operator H: V x R --. W is analytic and H(O,£) = O. The Jacobi
equation (103) can be written in the form
H (tp, c)1I = pll, h E  Il e (Ii. (106)
(II) The inhomogeneous linearized problem. The linearized equation
H.(O,O)II = h, h e  b e w: (107.)
corresponds to the boundary value problem
- h N - Pcrilfn(O)h = b,
11(0) = 11(/) = O.
( 107)
We have:
(i) For b = 0, problem (107) has the simple cigcnsolution hl(t) =
sin(7tt/I).
(ii) For b E  problem (107) has a solution iff
(blh.) = O.
where (vi ",) = J vw dt.
Hence the operator H.(O,O): V -. W is Fredholm of index zero.
(III) The generic branching condition. Letting b = - fN(O)h l , we obtain
(b I hI) :F 0, i.e., problem (107) has no solution. This implies the branching
condition
R(H.,c(O,O)h.) , R(H(O,O».
Observe that H(tp,t:) = -cp" - (Peril + £)/'(q», and hence HftC(O.O)h l =
- fN(0)h 1 .
Now, assertions (a) and (b) above Collow immediately from Theorem
8.A.
(IV) Computation of the nontrivial solution. According to Theorem 8.A., the
nontrivial solution branch has the form
cp = sh l + s2h2 + '.'9 P = Peril + tiS + t2S2 + ..., (108)
with (II I IliA) = 0 for all k > 2. Substituting this into the Euler equation
(102), a comparison of the terms with S2 yields:
- h 2 - Pc:ri,!"(0)h 2 = b, h 2 (0) = hz(/) = 0,

704
29. Noncoerci\'e Equacions and Nonlinear Fredholm Alternatives
where b = £If''(O)h l . From (blh) = 0 it follows that £1 = O. Further-
more. since (11.111 2 ) = 0, \ve get h 2 = O.
A comparison of the terms with S3 yields:
- h J - Peritf"(O)hJ = b, 11)(0) = 11)(/) = 0,
v,'here b = £21"'(0)11 1 + Pr'cfC4)(0)IIj6. From (blh 1 ) = 0 \ve get 82'
(V) Stability of the trivial solution. Letting cp = 0 in the Jacobi equation (103)
v,'ith P = Perit + £, we obtain
/Jmin = - f"(O)r.,
/"(0) > o.
Thus, the trivial solution cp = 0 is stable for £ < 0 and unstable for t > O.
(VI) Stability of the nontrivial solution. By Theorem 8.E, the smallest cigen-
\'alue Jlmin(') of equation (106) along the nontrivial solution «(,O(s), £(s») in
V x R has the form
Jladn(S) = 2£2 S2 + 0(S3), S -. O.
Note that (106) corresponds to the classical Jacobi equation (103). Since
Iladn(S)  0, for small .  0 if 1:2  0, we get the assertion (e). 0
29.13c. Explicit Solution of the Beam Problem
Proposition 29.49. For ever}' a E A, tlae ilJitial value problem
" P. 0
cp + A sin cp = ,
(109)
<,?(O) = 0,
cp'(O) = a,
litis tI rlniqrle global solut;oll on R.
f"'or a = 2k y'PjA ,,'ith 0  k < I, the solution of (109);s gil:ten b}'
q)(T) = 2 arcsin ( k sn ( ft T. k) ) for all T e R. (110)
The fu ncti on cp = q>(r) has the same zeros tIt as the elliptic function
T...... sn( J P/ A t. k). and hence
t" = 2,. fl K(k
n = O. :I: 1, :t: 2. . . . .
where
f. ./2 d. 11
K(k) = Y'
o y' l - k 2 sin 2 t/J
EXAMPLE 29.50 (The Beam). By Proposition 29.49 each solution of the Euler
equation (98) for the beam is obtained from (110) by letting lp(/) = O. Thus,

29.13. I\pplication to the Buckling of Beams
70S
'1
It +
2
(
rr = 9' (T)
. T
(a) t1 = .fn(. k)
(b) cp(O) = q;(/) = 0
Figure 29.15
I = tIt' and hence we obtain for the corresponding outer force
p = 4n: A K(k)1.
I
Note that the function k.-. K (k) is monotonically increasing and K (0) = 1[/2.
More precisely, we obtain
P = P,,(I + 8k 2 + O(k4)
as k  0,
where Pn = n 2 f[2 A/12. By (110), bifurcation occurs for k = 0 and hence for the
critical forces P = Pn, n = 1,2,... · Note that Perle = Pl'
The functions sn(') and cp(') are pictured in Figure 29.1 S. Both functions
arc periodic. The behavior of cp = cp(t) can be understood best if one observes
that the beam equation (109) describes the motion of a pendulum as well
(Fig. 29. 16(a». In the case of a pendulum, we have: qJ = angle, t = time.
m = mass, L = length. 9 = gravitational acceleration, and in (109) we have to
set P / A = g/ L. The larger a is in (1 09 the larger is the initial velocity of the
pendulum. If a is sufficiently large, i.c., if k > 1, then the pendulum completely
surrounds the circle in Figure 29.16(a). Such solutions lead to strange forms
of the beam, like those pictured in Figure 29. I 6(b), which may correspond to
a mctallic rod.
L
(a)
(b)
Figure 29.16

706
29. Noncoerci\'e Equations and Nonlinear Fredholm Alternatives
PROOF OF PROPOSITION 29.49. If a solution qJ = qJ(r) of(I09) exists on an open
interval t then <p is bounded, since sin(.) is bounded. Thus, the existence of a
unique global solution follows from the continuation principle in Section 3.3.
We want to show that cp in (110) solves equation (109). For brevity we set
PIA = 1. It follows from (110) that
sin qJ/2 = kt1 t (111)
where O'(t) = sn(t. k). Hence cos tp = I - 2Jc2(J'2. Differentiation of (111) yields
,,,
cp · cas 2 q> = k 2 q,2 = k 2 (1 _ k 2 (2)( I - (2)
4 2
= k 2 ( cos 2  )(I - (2).
Therefore. cp' 2/2 == cos tp + const, and hence
(cpN + sin tp)tp' = o.
Moreover. from (111*), we get q>'(O)/2 = kcn(Otk) = k.
(111 *)
o
29.14. Stationary Points of Functionals
By definition, the problem
F(u) = stationary!. u e S.
corresponds to the determination of all the stationary points of the functional
I:: S .-. R in the sense of Definition 29.51 below. For example, if S is an open
interval in Rt then u is called a stationary point of F iff F'(II) = 0, i.e., the
tangent of the graph of F is horizontal (Fig. 29. 17(a». The following definition
generalizes this situation.
Definition 29.51. Let f": S ..... R be a functional on a subset S of a real B-space,
and let Uo E S. We set
"'(I) = F(u(I)
where t is a real number. By an admissible curve through the point "0' we
understand a map u: U(O)  R .... S such that u(O) = U o and the derivative u'(O)
exists (Fig. 29.18). The point "0 is called a stationar}' point of F iff
tII'(O) = 0
for all admissible curves through 110.
EXA"IPLE 29.52. Let S = {(.,,) e 01 2 : 2 + ,,2 = I}, and let F(,,,) = 'I. Then
the function F: S -t iii has the two stationary points (0, :t 1) (Fig. 29. I 7(b».

29.14. Stationary Points or Functionals
707
F

(a)
(b)
Figure 29.17
PROOF. We set .p(t) = F((t), ,,(t». If  = (t), " = ,,(t) is a differentiable curve
on S with (O) = 0, '1(0) = + I, then 1/1'(0) = ,,'(0) = o. 0
Note that (0, + I) is Plot a stationary point of F: R2 -+ R, where again
F(t") = '1.
Proposition 29.53. Let F: U(u o ) s; X -+ R be a filnctional 0'1 a 'Ieighborhood of
the point Uo in the real B-space X. Then:
(a) If Uo ;s a stationary point o.f F, then
bF(uo; h) = 0
for all heX.
(b) Let F be F-differentiable at "0. Then U o is a statioPlar)' point of F iff
F(flo) = o.
Stationary points of functionals are frequently also called critical points. By
Proposition 29.53(b), this convention is meaningful with respect to the more
special definition of critical points for maps given in Section 29.10.
PROOF. Ad(a). Set I/I(t) = F(uo + th), where hEX. If Uo is a stationary point
of F, then ""(0) = c5/:(u o ; h) = o.
Ad(b). Let F'(flo) = 0, and let U = U(I) be an admissible curve through U o .
Letting .p(t) = 1'.(II(t»), we obtain that 1/1'(0) = F'(fl(O»U'(O) = 0, since u(O) =
flOe Thus, Uo is a stationary point of F.
Conversely, if "0 is a stationary point of F, then it follows from (a) that
F(uo' 12) = F'(uo)h = 0 for all hEX and hence F'(u o ) = O. 0
"0

Figure 29.18

708
29. Noncoera\'c Equations and Nonlincar Fredholm Altcrnati\"Cs
Corollary 29.54 (Stationary Points on Planes S). Lel F: U(uo)  X -. R be
F-differel,'iable at Uo. Let S = a +  \vhere Y i.'i a linear SUbspclce o.f X and
a E X. Let 140 e S. The" U o is a statiolwr}. point of F: S -+ R iff
c5F(uo; h) = 0 .for all heY.
( 112)
PROOF. We set .p(l) = F(U(I). Let Uo be a stationary point of F: S -+ R. Let
hEY. Then u(t) = Uo + th is an admissible curve through "0' since "(1) e S for
all t E R. From 4,lI'(O) = 0 we obtain (112).
Conversely, suppose that (112) holds. Let 14: U(O) s R -. S be an admis-
sible curve through "0. Then u'(O) E Y. By (112). 1/1'(0) = f'(uo)u'(O) =
bf"(uo; u'(O) = O. 0
29.15 Application to the Principle of
Stationary Action
The integral. considered in the principle of least action. can never have a
maximum, as Lagrange mistakenly believed; in no way. however. will it always
ha\'e a minimum.
Carl Gustav Jacob Jacobi (1837)
We consider the motion q = q(t) of a point or mass m on the real line. i.e.. 1
and q(t) are real numbers. Let U: IR -. R be a C 1 -function which we regard as
the potential energy of the mass point. The principle of stationary action reads
as follo\vs:
J. T ( )
dcr nJ .
F(q) = 0 "2 q'2 - U(q) dt = stationary!.
q e S,
( 113)
where
S = {q e C 1 [O T]: q(O) = a,q(T) = b}.
Here, at b, and T are fixed real numbers.
Proposition 29.55. The C 2 -fullcl;on q( · ) ;s a solution of (113) iff
mqH = - U'(q), q E S. (114)
Equation (114) is called Newton's equation of motion. Here, - U'(q) cor-
responds to the force acting on the mass point. This \\'ill be considered in
greater detail in Chapter 58.
PROOF. We set X = C 1 [0, T] and Y = {q e X: q(O) = q(T) = O}. For all hE
Y r. C 2 [0, T], integration by parts yields

29.16. Abstract Statical Stability Theory
709
F(q;lJ) = f: (mq'h' - U'(q)h)dr
= - faT (mqN + U'(q»h dr.
By Corollary 29.54, q is a solution of (113) iff tSF(q; h) = 0 for all heY: Since
the set Y n C 2 [0, T] is dense in Y. this is equivalent to
bF(q;/a) = 0 for all h e Yn C 2 [0, T]
and, in turn. this is equivalent to the Euler equation (114).
o
Corollary 29.56. A 5011liion q of tile Euler equation (114) can never be a local
maxitlUJI point of (113).
PROOF. Otherwise. q is a local minimal point of - F(q) = min!, q e S. By the
Legendre condition in Section 18.17b,
L ifif S 0,
where L =  q,1 - U(q but this is impossible.
o
The following example shows that nor all the solutions of the Euler equation
(114) are local minimal points of(113).
EXAt.tPLE 29.57. We set U(q) = w 2 q2/2 for fixed (J) > O. Then the Euler equa-
tion (114) describes the motion of a harmonic oscillator. The corresponding
Jacobi equation
-h" - w 2 h = ph, h(O) = h(T) = 0,
has the smallest eigenvalue Jtmln = (7t Z /T2) - w Z . From Proposition 29.47, we
obtain that:
(a) If T > 1[/0), then no solution of the Euler equation (114) is a local minimal
point of (113).
(b) However. if T is sufficiently small i.e.. if T < n/w, then every solution of
(114) is a strict local minimal point of (113).
29.16. Abstract Statical Stability Theory
The following abstract model allows, for example, important applications in
nonlinear elasticity. Let ns(b) denote the number of solutions of the equation
Au = b,
U E S.
( II 5)

710
29. Noncocrcivc Equations and Nonlinear Fredholm Alternatives
Our goal is Theorem 29J below. We assume:
(H I) Let X and Y be B-spaces over K = R, C, The C 1 -operator A: D s; X  Y
is Fredholm of index zero on the open set D, where I S k S 00.
(H2) Let Dn.b be a subset of D such that
A'(u)h = Ot
u e DUlIb' heX,
implies h = O.
(H3) Let S be a subset of D stab such that A: S ..... y is proper.
In elasticity, the set DAub corresponds to (strongly) stable states u of the
elastic body, and b corresponds to the outer forces and to the displacements
of the boundary of the elastic body. In Section 29.19 we consider general
variational problems. There, we \\rill set:
D = {u: the strong Legendre-Hadamard condition holds at u},
Dlab = {u E D: the second variation is strictly positive at u}.
EXAMPLE 29.58. Let A: D s X --. X. be a ct-Fredholm operator of index zero
on the open subset D of the real B-space X, where 1 < k < 00. We set
Dab = {u E D: A is locally strictly monotone at u}.
Then assumptions (H 1) and (H2) are statisfied with Y = X.,
This follows directly from Definition 29.20.
Recall that the operator A: S  X. is injective on each convex subset S of
D,lab' by Proposition 29.22.
EXAMPLE 29.59, We consider the local minimum problem
F(u) - b(u) = min!, u e D. (116)
Let F: D c X -+ R be a ct+ I-functional on the open subset D of the real
B-spacc X, ",'here 1  k S 00. Suppose that F': D c: X ..... X* is Fredholm of
index zero. Let
D stab = {u e D: F' is locally regularly monotone at u}.
If we set A = r, then the assumptions (H 1) and (H2) above are satisfied with
Y = X*. Let S be a subset of DSUlb. Then, each solution of the Euler equation
(115) is a strict local minimal point of (116), by Corollary 29.40.
If S is convex, then A is injective on S.
Theorem 29.J. Assume (H I) through (H3), Let beY - A(oS (\ S) be gif.,'J.
TIJen:
(a) The "umber 'Is(b)  0 is finite.
(b) Tire jr,nction ns(.) is constant on each connected subset of the ope'J set
Y - A(aS r. S).

29.16. Abstract Statical Stabilit), Theory
711
Corollary 29.60. Assume (HI) and (H2). Let S be a subset of D...ab. Then:
(a) For eacl, U E D 8tab , the operator A ;s a local Ct.diffeonJorphism at 14.
(b) If A is il,ject;ve on S, thel' A: S -+ A(S) ;s a C t -difJeonlOrph;sm.
PROOF. Theorem 29J follows immediately from Theorem 29.F, since the
subset D'inl of Dlab is empty, according to (H2).
Corollary 29.60 follows from Proposition 29.17(i). 0
The following corollary is related to the important fact that the apparentl)'
special assumption (H3) for the operator A: D  X -. Y can be satisfied for a
large class of subsets S of DIb.
Corollary 29.61 (Properness). Aulne (H 1). Then:
(a) Tile operafor A is proper on each compac' subset S of DSUb.
(b) If C is a compact subset of Dstllb' t lle n there exist. all open neighborhood U
of C SIICII that A ;s proper on S = u.
(c) Let the B-space X be separable. If C is a bounded closed .wbset of D,lab-
tlJellfor each c > 0, there exists an open neighborllood U of C Silch ,IIal
dist(oU,cC) < £,
alld A is proper OIJ S = U (Fig. 29.19).
(d) If A ;s proper OIJ a set Do.. the" A ;s also proper on each closed Stlbset S of Do.
(e) If A is proper on a finite nlllnber of ..ubsets of D, tlJen A is also proper 01'
tile Iinion of these sllbsets.
Statements (b) and (c) arc especially important in connection with Theorem
29.J.
PROOF. Ad(a (d) (e). Note that closed subsets of compact sets are compact t
and the union of a finite number of compact sets is compact.
Ad(b). Let u e D.tab. By Corollary 29, 60, A is a local diffeomorphism at u.
Moreover, by Proposition 29.17(d), A is locally closed. Thus. there exists a
closed neigh borhood V of u such that A: V ... A (V) is a homeomorphism, and
the set A (V) is closed in Y. Hence A is proper on II:
c
D
Figure 29.19

712
29. Noncoerci\'e Equations and Nonlinear Fredholm Alternatives
Now note that the compact set C can be covered by a finite number of such
sets II:
Ad(c). Let {U 1 , U2'...} be a dense subset of C. Sct Crt = C" span{u 1 ,...,
II,,}. and apply the argumcnt (b) to C II for sufficiently large n. 0
29.17 The Continuation Method
Let Uo be a point in the domain of stability D 8t8b and let b = bet) be a
CI-curvc \vith Au o = b(O). where 1 is a real parameter. Since A is a local
CI-diffeomorphism at Uo, there exists a CI-curve II = U(I), for real I in a
neighborhood of t = 0, such that 11(0) = Uo and
A (U(I» = bet).
( II 7)
This solution curve can be continued as long as the curve remains in the
domain of stability D'lab' In elasticity, the curve u = u(t) corresponds to a
deformation process. Bifurcation may occur if the curve reaches the boundary
of stability oD fAab (cf. Fig. 29.11 (b». Differentiation of (117) yields A'(u(t))u'(t) =
b'(l). and hence
u'(I) = A'(U(t)-l b(t).
( 118)
Discretization of the time derivative u'(t) yields thc approximation method
v It + I = v" +  I A' ( v,,) -1 b' (nt), n = 0, I, . . . t ( 119)
where t'n corresponds to lI(nt) and Vo = Uo. The convergence of this method
will be proved in Section 61.6 in connection with nonlinear elasticity. This
proof proceeds completely analogously to the proof of the classical Peano
existence theorem for ordinary differential equations. The necessary com-
pactness is obtained through embedding theorems.
29.18. The Main Theorem of Bifurcation Theory for
Fredholm Operators of Variational Type
We want to prove that (0. 0) is a bifurcation poi,.t of the equation
H(U,A) = 0, u e X. i eA. (120)
where the parameter )..lives in the parameter space A. More precisely, we want
to show how to reduce problem (120) to a finite-dimensional variational
problem (Proposition 29.64 below). and \\'e will use this information in order
to prove an important bifurcation theorem for (120).
A pecrllarjl) of our approach is that we do not restrict ourselves to the case
of H-spaces as this is usually done in the literature. This allows us in Section
29.20 to pro\'c bifurcation theorems for variational problems "..ithout any

29.18. The Main Theorem of Bifurcation Theory for FredhoJm Operators
713
growth conditions, In this connection. we will use the B-spaces of smooth
functions
x = {v E C 2 '«( G ): V = 0 on oG},
and \\'e will set
y = (G), 0 < a < I,
«(II w) = fG VW dx for all v, w € Y.
Note that Y is nOI an H-space. The H-space approach corresponds to the
Sobolev spaces
x = Y = W 2 1 (G)
\vith the usual scalar product ('I') on X. In this case, one needs growth
conditions.
In (H3) below, we introduce a new class of operators which we call operators
of t'arialionall}'pe. These operators can be regarded as gelJeralized potential
operators. Roughly speaking, operators of variational type are "potential
operators" with respect to a generalized scalar product ('1') on a B-space 
In the special case of an H-space X with the usual scalar product ( 'I' ), we set
y = X. Then, operators of variational type and potential operators coincide.
This will be discussed below.
We assume:
(H 1) Let X, Y, A be real B-spaces such that the embedding X c Y is con-
tinuous. Let the operator
H: U(O,O) c X x A.... Y
be C" on a neighborhood of the point (0,0), where 1 < k < 00,
(H2) Linearization. Suppose that H(O, ;_) = 0 and that the linearized equation
H,,(O,O)h = 0,
he X,
has exactly n linearly independent solutions with n  1. Suppose that
H..(O.O): X -+ Y is Fredholm of index zero.
(H3) Variational structure. Assume that there is a .l-functional f:
U(O.O)  R such that the following key condition holds:
df(ll t i.; h) = (H(u, i.) I h)
for all heX. (u. i.) E U(O,O),
Here. f denotes the first variation of the functional f with respect to
the first variable u. that is.
5\ ( ,. . h) _ I " f(u + rh, l) - f( ))
UJ I U, I., - 1m
rO t
and (u, V) 1-+ (III v) denotes a given bilinear, bounded, symmetric, strictly
positive functional from Y x Y to R.
We say that the operator u.-. H(II, 1) is of variational type iff the condition
(H 3) is satisfied.

714
29. Noncoercive Equations and Nonlinear Fredholm Altcmati,'cs
(H4) Generic branching condition. Suppose that the F-deri,'ative H...t(OtO)
exists and that there is a special parameter i. 1 such that
(l'IHuA(O, 0)VA 1 ) :/;- 0
for all (' e N(H..(OtO» with v :F O.
(H5) Remainder. Let
R(u,l) = H(U,A) - Hu(OtO)u - H...t(OtO)lti.
and suppose that
II R(u, ).) it  const IIIIn 2
for all (u, A) in a sufficiently small neighborhood of (Ot 0).
The following assumptions will be used in Corollary 29.63 below, but
not in Theorem 29.K.
(HS*) Special remainder. Define R(') as in (H5). Suppose that
R(u,eA 1 ) = SI4 + eTr4
for all (u, e) in a neighborhood of (0, 0) in X x (R, where the operators
S, T: U(O) s X -+ Yare C' and S(O) = T(O) = O. S'(O) = T'(O) = O.
(H6*) The operator v...... H.,).(O, O)VA 1 transforms the null space N (Hy(O, 0»
into itself.
EXAMPLE 29.62. Let ).. be a real parameter, and suppose that
H(u, i.) = Lu + ,tAil + SI4 + ATu, (121)
y,'here L, A: X .... Yare linear continuous operators t and S, T: U (0) e X..... Y
are C", 1  k S IX) with S(O) = reO) = 0 and S(O) = T'(O) = O. Then
H,,(O,O) = L, HuA(O,O)u). = ;.Au,
and conditions (HS), (HS*) are satisfied. Moreover t in the important special
case
Au = u
forall ueX t
the generic branching condition (H4) and hypothesis (H6*) are satisfied triv-
ially with i... = I.
If X is an H-space with scalar product ( '1' ), then we set Y = X. In this case,
condition (H3) is satisfied iff
(Hu('t, )/Jlk) = (Hu("t )kIIJ) for all h, k e X,
and all (14, ).) in the convex open neighborhood U (0. 0). The functional f:
U(OtO) £ X x A .... (R is then given by
f(u, i..) = fo1 (H(tu, i..)lu)dt.
This follows from Proposition 41.5. In this special case, operators of varia-
tional type and potential operators coincide.

29.18. The Main Theorem of Bifurcation Theory for Fredholm Opcracors
715
"t
,\
(a) m = 2
(b) m = odd, m  4
fe"igure 29.20
(c) m = even
Theorem 29.K. If (HI)-(HS) hold ,,'ich k  2, then (0,0) ;s a bifurcation point
of tI,e equation H(u,).) = 0, u e X,). e A.
More precisely. the bifurcation takes place in tile direction of the paranleter
). J , i.e.. the point II = 0, t = 0 is a bifurcat;olJ point of the equation H (u, £Aw 1 ) = O.
II e X, I: e R.
The prototype for this theorem is given by the real equation
- AU + u'" = O. u, l e R. m  
\vith the trivial solution 14 = 0, l = arbitrary, and the nontrivial solution
A = U.,.-1 (Fig. 29.20).
Recall that n is the number of the linearly independent solutions of the
linearized equation Hy(O,O)1I = 0, heX.
Corollary 29.63. Assume (HI)-(H4) and (HS*), (H6*), and a5SllnJe tllat
H( - u. ).) = - H(u, A) for all (u, A) e U(O, 0).
Tlle'l there exist n "solutio'J branches" in a neighborhood of (0,0) in the direction
of tile paralne'er AI.
More precisel}', there exists a fanJ;ly of (n - I )-di,nensional Clc-manifolds Jr
Figure 29.21

716
29. Noncoerave Equations and Nonlinear Fredholm Alternatives
ill the IUIII space N(H.,(O.O» suela that, for each r E ]0, ro[, the follo\v;lIg hold
(Fig. 29.21):
(a) M,;s C"-difJeomorphic 10 a sphere of radius r in [RII, 0, JWf1 and
max{ttull: u e kl,} -.0
as r -. O.
(b) J\{, contains II pairs {IIi" - Uir} alld there are real numbers £ir such that
H( :t Uir' £irA,l) = 0,
altd ( + "tI, 6u,4.l) -. (0,0) as r -+ 0 for all i.
The proofwiU be based on Proposition 29.64 below. Since dim N(H.,(O,O» =
la, there exist elements VI' . . ., VII of X such that H.(O,O)Vi = 0 and
(vilvj) = b lJ for i,j = I, ..., n.
i = 1, ....I
For all u e y, define
"
Pu = L (villl)v;.
.=1
Then the operators P: Y -. N(H.,(O, 0» and P: X -. N(H.(O, 0» are linear con-
tinuous projection operators onto the null space N(H.,(O,O», since the em-
bedding X  Y is continuous. We set
pl. = 1 - P.
Observe the important fact that, with respect to ( .1.), the operator P has the
same properties as an orthogonal projection operator on an H-spacc, i.c., we
have pz = P and, for alii., keY,
(Phlk) = (/JIPk), (PhIP 1 k) = O. (122)
We now use the method of the branching equation of Ljapunov which we
have carefully studied in Section 8.6. Letting u = v + ,v, the original equation
H(u,l) = 0, u e X, A e A, (123)
is equivalent to the system:
pi H(v + \\', A) = 0,
PH(v + "', A) = o.
vePX, ,,'ep1X,
( 124)
(124 *)
The following proposition tells us the crucial fact that the solution of the
branching equation corresponding to (124*) can be reduced to the solution
of a finite-dimensional variational problem. This is the key to our proof
below.
Proposition 29.64. (Reduction Trick). Assunle (HI), (H2), (H3). Theil:
(a) There exists a C"1unction \\' = w(v.).) on some I.eiglJborhood of (0,0) in
pl. X x A such that all the solutions of (124) have the form (v + w(v, ).),)
in a sufficientl}' small neighborhood of (0,0) in X x A.
(b) ,\.'(0, ).) == 0 and \\.'&7(0,0) = O.

29.18. The Main Theorem of Bifurcation Theor)' for Fredholm Operators
717
(c) Set
(,O(V, A) = f(v + ,v(v, A.), i,,),
v e P X, ). e A.
There e",=iSIS a neighborhood V of (0,0) in X x A such that the point (u, )..) e V
is a solutio'1 of the original equation (123) iff u = t' + ",(v, i,,) and t' is a
Crilic(ll point 0.( cp, i.e.,
tpt'(V, l) = 0,
vePV. leA.
(d) If. in addition. condition (HS) holds, tl,en the fUllctional tp lias the form
(,O(v,.() = !(vl H.,)JO, O)vi.) + r(v, )..),
",here r( L'.).,) = o( II v n 2). t' -+ O. uniforml}' ,vilh respect to all i.. in a srljJicienll)
smallneighhorlJood of )" = 0 in 1\.
PROOF. Ad(a). We proceed analogously to Section 8.6.
(I) The range of Hy(O, 0). Let b e  We show that the equation
11..(O.O)v = b,
veX.
( 125)
has a solution iff (bl Vi) = 0 for all i, i.c., Ph = 0. Hence R(H.,(O, 0) =
pl Y. Consequently. the operator pl H.(O, 0) is a linear IJonleomorpllis",
from pi X onto pl t:
Indeed. it follows from (H3) that b 2 f(0, 0; h, k) = (Hy(O, 0)11 Ik), and hence
(H..(O,O)lJlk) = (Hy(O,O)kI/J)
for all IJ, k e X,
since b 2 f is symmetric. We now construct Ii e y* through (/;, v) = (vii v)
for all.., e Y. Recall that Hy(O,O)v i = O. Thus,
(!t.ll..(O,O)v) = 0
for all 1i e X,
and hence j, E N(H.,(O, 0)*). Since H,,(O,O) is Fredholm of index zero,
dim N(H.,(O, 0)*) = dim N(Hy(O, 0») = II (cf. Section 8.4). Consequently,
.(H.,(O, 0)*) = span {II' . . . , f,.}, and hence equation (125) has a solution iff
(j;,b) = 0 for aU i.
(II) Solution of (124). Letting F(u,)..) = P 1 H(u,A), we obtain F..(O.O) =
pI Hy(O, 0). By (I), this operator is surjective from X onto pl.  Thus,
assertion (a) follows from the surjective implicit function theorem
(Theorem 4.H).
Ad(b). From H(O, )..) = 0 and (a) we get w(O.,i) = O. Letting ", = ",(ti, i..),
differentiation of (124) with respect to t" yields
p J Hy(O,O)(h + "',.(O,O)h) = 0 for all h e P X.
Hence
pl HyCO, 0)'''.,,(0, O)h = 0 for all h e P X,
where ,,,.,,(0,0)11 E p.l. X. By (I), ,,'&,(O,O)h = O.

718
29. Noncoerci..-e Equations and Nonlinear Fredholm Alternativcs
Ad (c). Substituting)\) = w(v, A.) into (124), we obtain
pi H(v + ",(v, ).),).) = O.
(126)
Thus, it is sufficient to prove that the equation lp,,(v,).) = 0 is equit'alent to the
branclaing equation
PH(v + w(v,i.)i.) = O. (126-)
In fact, noting (H3) and (126), this follows from
cp,,(v, ),)/1 = (H(v + ",(v, l) l)(h + wil(v, l)h)
= (P H(v + w(v, ).), ;')Ih + w,.(v, A)h)
= (PH(v + w(v,).)l)lh) for all he PX.
In this connection. observe Pw(v, A) = 0 and (122).
Ad(d). From (b), we obtain
w(v. i.) = ",(u. ).) - ",(0, J.) = fal W,,(w, A)V r.
and hence
w(v, A) = o(lIvll), v -+ 0,
uniformly with respect to smallliAIi. By (H3
feu, i.) = fol (H("', J.)/ u) dr,
The assertion now follows from (H5). In this connection, note that H,,(O.O)v =
o for all v e P X and
I(HuA(O,O)l\\'(v,i.)A.lv + )\)(v,A»1 S IIH".t(O, 0) II 1I'\\'(v,i.)1I IIllI(lIvll + IIw(v,j)II)
= o( II V 11 2 ), V -. o. 0
PROOF OF THEOREM 29.K. We set
a(h, k) = (HuA(O,O)hA1Ik) for all la, k e X.
Let (O,l) e U(O,O). The symmetry of 6 2 fyields
(H.,(O, i.)/llk) = (H.,(O, l)klh) for all h, k E X.
Differentiation with respect to l implies the symmetry of a( ., .). Moreover,
a( .. · ) is definite on P X x P X according to (H4). Letting
A = tAl for real £,
the assertion follows immediately from Propositions 29.2S(H) (iii) and 29.64.
o
The proof of Corollary 29.63 wiU be based on the following simple '.multi-
plicr trick."

29.18. The Main Theorem or Bifurcation Theory for Fredholm Operators
719
Lemma 29.65. Sr4ppose that we are given operators A, B: D 5 X   points
u e X, v e P X, z(h) e pi X, and real numbers t;(v), p such that the follo\v;IJg hold:
Pl.(Au + e(v)Bu) = 0, (127)
(Au Iv) + t(v)(Bulv) = 0, (128)
(Aulh + z(h» + Il(Bulh + z(h» = 0 for all h E PX, (129)
and (Brllv + z(v» #; O. Then u is a solution of the equation
Au + e(v)Bu = o. (130)
PROOF. From (127) and (128), we obtain
(Aulv + z(v» + £(v)(Bulv + z(v» = O.
By (129), JJ = e(v). Hence, by (127) and (129),
(Aulh) + £(v)(Bulh) = 0 for all II e P X,
i.e., PAu + t(v)PBu = O. From (127) we get (130). 0
PROOF OF COROLLARY 29.63. By (HS$),
H(U'£l) = Au + e8u,
where
Au = Hu(O,Q)u + Su and Bu = lIIlA(O,O)UA) + Tu,
and Sri, Tal = o( II 141:). "U II -+ O. By (H 3),
f(u, eA. 1 ) = a(r4) + tb(u),
where
a'(u)h = (Aulh)
Finally, we set
and
b'(ll)h = (Bulh)
for all heX.
u = v + w(v,t(v);..)
and
a(v) = a(u),
P(v) = b(u).
(I) The basic ideas. The following considerations will be justified below.
Ad(127). By (124) and Proposition 29.64, equation (127) is valid for each
small real number t(v).
Ad(128). We fix t(v) in such a way that (128) holds.
Ad(129). We consider the problem
(X(v) = stationary!, P(v) = r 2 , v e  (131)
where V is a fixed sufficiently small neighborhood of v = 0 in P X, and the real
number r > 0 is sufficiently small.

720
29. Noncocrci...c Equations and Nonlinear Fredho1m Alternatives
Let I' be a solution of (131). By the Lagrange multiplier rule (cf. Section
43.10), there is a real number p such that a' (v) = - Jl!J'(v), i.e.,
'(v)h + pfJ'(v)h = 0 for all II E P X.
This is equation (129), since
a'(v)h = (Alii II + \\'&,h + r).t'(v)h),
{J'(v)1I = (Bulh + \v/1 + \v.(t'(v)h) for all h E P X,
( 132)
where \\' = \\'(v, ';(V)A 1)'
Equation (130) is our original equation H(U'£;'I) = 0. Therefore, we are
done if we can justify the following:
(i) We can solve equation (128) for e if II (ill is small.
(ii) The finite-dimensional variational problem (131) possesses n pairs of
solutions (v, - v).
(iii) The solutions of(131) have the property (v,e(v».-. (0,0) as r -+ O.
(iv) We can use the Lagrange multiplier rule from Section 43.10, i.e., we have
{J'(.J)I.' i: ° for P(v) = r 2 .
(v) The set {v e V: !J(l') = ,2} is C" diffeomorphic to a sphere for small, > O.
In the following, U o ( II vII"), v  0," always means "uniformly for smalllel. n
(II) Justification.
Ad(i). Differentiation of(124), p1 H(v + \v(v,eAl),tl) = 0, with respect to
e yields
(D) (pi Hu(O, 0) + C)\V 1 = - pi HuA(O' O)WA 1 - pI T(t' + \\.),
where
C = tPJ.H w1 (0,0»).1 + p.lS'(u) + tP1T'(u)
and II = V + w(v, £i. 1 ). In this connection, observe the important fact that
pl H..1(O,O)vl l = 0
by (H6*). Rccall that
\v(v, eA I ) = o( II vII), v -+ 0,
and Su, Tu = o( lIull)' u  O. Since p.1 H..(O, 0) is a linear homeomorphism from
pl. X onto pi Yand IICU is small for smallllvH, we obtain from (D) that
II "r;.11 < const Ii (pi H,,(O, 0) + C)-I II ( II \\. II + o( II v + \' II ).
Hence we get the ke}' relation:
"';.(li, tl.) = o( II vII ). v  0.
Equation (128) has the form
(E)
W(V, v) = -(Sulv) - e(Tulv),
V E P X, I; e R,

29.18. The Main Theorem of Birurcation Theory ror Fredholm Operators
721
with II = V + ",(v, t).I) and
a(t" v) = (H"l(O. O)vl. 'v).
No\v note the decisive fact that a(., .) is definite. according to the generic
branchi"g condition (H4). Without any loss of generality, we may assume that
a( ., .) is pos;tilte definite on P X x P X.
In Problem 29.8 we prove a simple variant of the il,aplicit funcliolllheorem.
This result sho\\'s that there is a neighborhood W of the origin in P X such
that equation (E) has a Ct.solution £ = t:(v) in IV - {O} and
t(v)  0 as v.... o.
In this connection, note that Sri = o( UrlII) and Tr4 = o( !lu,I). u ..... 0, and
,vl(v, f:A. I ) = o( IIvll) as v -+ o.
Ad(ii). Differentiation of (E) with respect to v yields
£'(v)v = o( I), v -+ o.
By (132),
fJ'(v)v = a(v, v) + o( II vII 2), V ..... 0,
fJ(v) = fol (B(III)lu) dl = ta(v, v) + o( IIvll Z).
Recall that II = It + ",( rJ. tl l ).
We no\v come to the point of our proof. The finite-dimensional variational
problem (131) has always at least one solution (e.g., a minimum). This way
we again obtain Theorenl 29.K. However, if H is odd, then f is even and
'" = \\'(v, i.) is odd with respect to v. Thus. tX(.) and P(.) are et'elJ. In this case,
the Ljuslerllik-Schnirelman theory tells us the deep result that problem (131)
has II solution pairs (v, - v), since dim P X = n. This result together with impor-
tant generalizations to B-spaces will be proved in Chapter 44 (cr. Theorem
44.8).
Ad(iii), (iv). This follows from (133).
( 133)
Figure 29.22

722
29. Nonooercivc Equations and Nonlinear Fredholm Alternatives
Ad(v). Passing to an equivalent scalar product on PX, if necessary, we
can assume that a(v, ".) = (vi \v) for all v, ". E P X. Let v e P X be given with
2- 1 (vrv) = ,2 for small, > O. We now consider the equation
fJ(tv) = ,2 t
t E R.
( 133*)
Letting t = 1 + I: for small I: and using (133), it follows from the im-
plicit function theorem (Problem 29.8) that equation (133-) has a locally
unique solution t(v) near t = 1. The C*-map VH t(v)v yields the desired C".
diffeomorphism (Fig. 29.22). 0
29.19. Application to the Calculus of Variations
Let us study the variational problem
JG L(x,u(x),u'(x»dx - JG K(x)u(x)dx = min!,
" = 9 on iJG.
Our main goal is to obtain sufficient conditions for local minima. We write
problem (134) in the form
f(u) = min!, U E 9 + X, (134*)
( 134)
and assume the following:
(H 1) Let G be a bounded region in R N with oG e CO. I , and let
U = ("1' . . . , u", ),
x = (1'. · · ,  N ),
where N, M  1. We set D i = alai. Then, the f-derivativc u'(x) cor-
responds to the matrix (DiUJ(x» with i = I, . . . , Nand j = I,..., M.
(H2) Let the function L: G x DiAl X RM. -+ IR be CrS>, and let
K e L 2 (G)M, 9 e C 2 ( G )'v.
As usual, we set Ku = L11 KJu J .
We now introduce the following spaces:
X = {u e C 1 (G)-": u = 0 on oG},
Y = W 2 ' (Gt', Z = Lz(Gt'.
Recall that, by definition, the product space W. W consists exactly of all the
tupels ". = (WI'. . ., w.w) with "J E W for all j. The norm on W'" is given by
.v
II M'II WAf = L It "j Ii w.
)=1
For example, in elasticity, we have N = M = 3, and the quantities allow

29.19. Application to th Calculus of Variations
723
the following physical interpretation:
u = displacement of the elastic body,
L = density of the elastic potential energy,
K = density of the outer volume forces.
9 = displacement of the boundary of the body.
In the following, we Slim over two equal indices, where ;, j = 1,..., Nand k,
m = I,..., M.
29.19a. The Euler Equation and the
Legendre-Hadamard Condition
Proposition 29.66 (Necessary Conditions Cor a Local Minimum). Assume (H I),
(H2). Let II e C 2 (G)M be a local mininaal point of the original problem (134*).
The" u satisfies tile Euler equation
-div L..,(x, u(x), u'(x» + Ly(x. u(x), u'(x» = K(x) on G,
u = 9 oPJcG.
.'v/ oreover, tile second variatioPJ {)2f is positive, i.e.,
6 2 f(u: h)  0 for all h e CO(G)M,
where q = (x, u(x), u'(x» and
(,2f(u; h) = fG L.,....(q)h'2 + 2L.".,(q)h' h + L.,.,(q)h 2 dx,
a"d the Legendre-Hadamard condition is valid, i.e.,
L.".,.(x. u(x). u'(x»(d 0 V)2 > 0
for all x E G, d eRN, v e (RM.
Corollary 29.67. If u e C 2 (G)'" is a solution of tlae modified problem
fG L(x, u(x), u'(x»dx - fG K(x)u(x)dx = stationary!,
u=g onoG,
tllen u is also a solution of the Euler equation (135).
Explicitly, the Euler equation (135) reads as follows:
( CL ) oL
- D j aD + -;- = K" on G,
,II. uU"
U" = 9t on oG, k = I...., M.
( 135)
(136)
(137)
( t 35*)

724
29. Noncocrci\'C Equations and Nonlinear Fredholm Alternatives
Furthermore. if we set
( ) _ 0 2 L(x, Il(x), u'(x)
a ikj'" x -  D  D '
(/ I"" (i J U '"
then L"...h,2 = U.il",Dlh"DJh"" and the Lege,ulre-Hadamard condition (137) is
identical to
CliAJ",(.,'\:)diVidjV",  0
PROOF. Let hEX. We set
q>(t) = f(u + lh), t e R.
for all x e G, d eRN, v E R"'.
Then the real function qJ has a local minimum at t = 0, i.e.,
cp'(O) = 0
and
q>"(0)  o.
Integration by parts yields
o = q>'(O) = fG (L..h' + L.h - Kh)dx
= fG (-div L..- + Lu - K)h dx for all hEX.
This implies the Euler equation (135).
Since 02f(u; h) = q>'(O), we obtain (136) from cp"(O)  O.
Finally, the Legendre Hadamard condition (137) follo\\'s from Section
18.1 7b.
Corollary 29.67 follows from tp(o) = o. 0
29.19b. Sufficient Conditions for a Strict Local Minimum
and Strongly Stable Solutions
Definition 29.68. Let u e C 2 (G)M. Then u is called slro1lgly stable iff
t5 2 f(u;h) > 0 for all h e'y - to}, (138)
and the so-called strong Legendre- Hadamard condition is valid, i.e..
L.....,(x, u(x), u'(x»(d 0 V)2 > 0, (139)
for all x e G and all nonzero d eRN, v e R'''.
Theorem 29.L. Assume (H 1), (H2). Let U E C 2 ( G )'" he a strong I)' stable solutiO'J
of tile Euler eqllatiolJ (135). Tlle'J U i. a strict local minimal point of the origi'lal
proble,n (134.).
PROOF. Let Co be the minimum of the functional
"'(x, d, v) = L.....(x, u(x), 11'(x»)(d 0 1,)2

29.19, Applicacion to the Calculus of Variations
725
on the compact set {(X, d.I') E G X 1Ji.'1+M: Idl = Ivl = I}. Then Co > 0 and we
obtain that
Lu'"'(x, u( 14'(x»(d 0 V)2 > c o ldl 2 lvl 2 , (140)
for all x e G, d e (Ii''', v e R M . Explicitly, this means
auJ...(x)d,l'tdJ1'm  coldl l lvl 2 for aU x E G , d E IRN, v e AM.
( 140* )
By Problem 22,7b. the strong Legendre-Hadamard condition (140) implies
the decisive GdrdiPlg iPleqrlalil }':
c5 2 f(u: h) > c II/III - ClJl'II. (141)
for alllJ E Y and fixed numbers c > 0, C  O. Hence the second variation b 2 f
is regular at 14.
In fact, the assumptions of Problem 22.7b are satisfied since integration by
parts yields
b 2 f{r,;h) = fG hJ(u)h dx
for all h e C:( G)'v t
where J(u) denotes the so-called Jacobi differential operator. Our discus-
sion after (142) below shows that the strong Legendrc- Hadamard condition
implies the strong ellipticity of J(u) which we need in Problem 22.7b.
From (138) and (141) it follows, by the Hestenes theorem (Proposition
22.39), that
c5 2 f(u;h) > Ca rhll for all heY and fixed Cl > O. (141*)
The assertion now follows from Theorem 29.1 and the reduction trick in
Remark 29.33. 0
29.19c. The Jacobi Equation and the Eigenvalue Criterion
We set
/Jmin = inf c5 2 f(u; h),
lieS
where S = {It e Y: (IJ I IJ)z = I}. Moreover, we consider the so-called Jacobi
eqllal ;011
J(Il)h = ph on G,
h = 0 on cG,
( 142)
\vhere q = (x. r4(x), u'(» and
J(u)/1 = - div (L"'y(q)h' + LY'y(q)h» + L...,,(q)h' + LIIJI(q)h.
Explicitly. equation (142) means
-Dj(aiAj",D;h,,) - D j (L" 14 D)tl M h ,,) + LIIMD,,,..D;h,, + L"."Mhrc = Jh", on G,
II. = 0 on oG, m = I,.... M. (142*)

726
29. Noncoerci\'c Equations and Nonlinear Fredholm Alternati\'es
The principal part of this equation is given by
- Dj(aiii",Dfh,,) = - a'iJ'" DIDJh i + · · · ,
and (142) represents a linear strongly elliptic system in the case where the
strong Legendre-Hadamard condition (140*) holds.
Observe that the Jacobi operator J(u) corresponds to the linearization of
the Euler equation (135) at u.
Proposition 29.69. Assume (H 1), (H2). Let U E C 2 (G)M be a solution of tile Euler
equation (135), ,,,hich satisfies the strong Legendre- Hadamard condit ion (139).
TIJen:
(a) If I'mln > 0, tllen u is a strict local nJinimal point o.f the original problem
(134*).
(b) If I'min < 0, then u is not a local minimal point of (134*).
CoroUa!)' 29.70. In addition, let u e C 2 '«( G )-" and oG e C 2 ,01 for J;,ed « ,,,ith
o < a < 1. TI,e" the number J,lmin is equal to the smallest eigenvalue of tile
Jacobi equatio'l (142).
Furthermore, for all la, k e C:(G)M. we have
b 2 f(u;h.k) = fG kJ(u)hdx. (143)
Relation (143) shows the natural connection between the Jacobi differential
operator J(u) and the second variation b 2 f.
PROOF. We set
a(h, k) = b 2 f(14; II, k)
for all h, k e 
.
I.e.,
a(h. k) = fG L......h' k' + L"...h' k + L....hk' + L...hk dx,
where the argument of L is (x, u(x), u'(x»).
The assertions now follow from Corollary 29.41. 0
PROOF OF COROLLARY 29.70. Integration by parts yields (143).
By Corollary 29.41, the number /lmln is equal to the smallest eigenvalue of
the equation
a(h. k) = Jl(hl k)z
(144)
for fixed hEY and all keY, where (hlk)z = IGhkdx. Noting (143 we obtain
(144) from (142) in the case where the functions are sufficiently smooth. Thus,
equation (144) is nothing other than the generalized problem to the classical
Jacobi problem (142). Now, the regularity theory for strongly elliptic systems
tells us that each generalized solution of (142) is also a classical solution (see

29.19. Application to the CaJculus of Variations
727
Section 61.11). In this connection, observe that u E C2'(G)'" im pli es that the
coefficients of the Jacobi equation (142) belong to the space (G). 0
29.19d. The Euler operator in Spaces of Smooth Functions
We write the Euler equation (135) in the form of the operator equation
All = (K,g),
U E X, (K,g) e 'W,
(145)
\\'here we set
!I' = C 2 '.(G)"',
'!IJ = ('I(G)'" x C2.CI(cG
and
D = {" E q-: u satisfies the strong Legendre-Hadamard condition (139)},
D.uabl = {u E: u is strongly stable}.
It follows from (140) and (141*) that D and Dstablc are open subsets of the
B-space .
For the following, it is very important that 0 <  < 1. We assume:
(A I) Let G be a bounded region in [R'v with oG e C 2 ,:a, where N > 1 and
o < 2 < I.
(A2) Let L: G x 1Ji' x R"'.v -t> IR be C.
The following deep result (b) is crucial.
Proposition 29.71. ASSllme (AI), (A2). The,l:
(a) For each (K,g) E '!II QtJd u e :!" tile linearized equation
A'(u)h = (K,g h e :1:,
(146)
corresponds 10 tile Jacobi equation
J(u)h = K on G,
h = g on aGe
(146*)
(b) The operator
A: D : -+ 
is C«'I and Fredholm of index zero.
(c) Let u E D'blc' If A'(u)h = 0, h E .'r, then h = O.
PROOF. Ad(a). From the second-order Euler equation (135) it follows that
A()  0/1. Linearization of(135) yields (146*).
Ad(b). Recall that the Jacobi equation (146*) represents a linear strongly
elliptic system if u e D. A deep theorem on such systems tells us that A'(ll): I ....
 is Fredholm of index zero (cr. Proposition 61.17 in Part IV).

728
29. Noncoercive Equations and Nonlinear Fredholm Alternatives
Ad(c). If II e r is a solution of (146*) with K = 9 = 0, then h = 0 on aG
and integration by parts yields
2f(u: h) = fG II.I(u)h dx = o.
Hence II = 0 by (138).
o
29.1ge. The Number of Strongly Stable Solutions of
the Euler Equation
We write the Euler equation (135) in the form
Au = (K,g), u E S,
( 147)
where S is a subset of Dstablc, and where (K, g) E .'!J is given. Let ns(K, g) be the
,ul"lber of .olut ions of ( 147).
Proposition 29.72. Assume (A I), (A2). T/,ell:
(a) For each II e Dlablc' the operator
A: D s;  -+ 
is a local Coo-diffeomorphism at u.
(b) Let S be a subset of Dstable Sllch lhal A is proper 011 S. Theil, for eacll
(K,g) e  - A(aS n S),
the number ns(K, g) is finite.
Furlhermore. IIS(.) is constant on each c01lnected subset of the open set
. - A(eS n S).
CoroUaf)' 29.73. If C is a compact subset of Dstable, thell tl,ere exists an ope"
set U ill Y such thaI
c c U c D... ab1c '
and A is proper OIl S = o.
If the operator A is proper on the silbsets St.. . ., Sit of Dablc' then A is also
proper on S = UtS"
PROOF. This follo\\'s from Proposition 29.71 and Section 29,16.
o
These results show that the Euler equation (135) has nice properties with
respect to smooth strongly stable solutions.
Remark 29.74 (Bifurcation). Proposition 29.72 tells us the important fact that
the local one-to-one correspolulence between the right-hand sides (K. g) e 
and the solution u e. of the Euler equation (135) can be violated merely

29.19. Application to the Calculus or Variations
729
at points
II E !r - DSlablc,
i.e., u is ,wt strongly stable. In this casc, bifurcation may occur. Since the set
D,.'ablc is open in Y. bifurcation may occur at the points
U E oD,tablc,
i.e., II belongs to the boundary of stability. In this connection, a typical example
has been considered in Rcmark 29.29 and Figure 29.11. We thus obtain the
following important general principle:
Loss of strong stability can lead to bifurcation.
29.19f. The Continuation Method
Let "0 e D.. ab1e be a given solution of the Euler equation (135), i.e.,
Auo = (Ko. go)'
In order to continue this known solution uo, we consider the equation
Au, = (I - t)(Ko.90) + t(K,g), u, E, (148)
\\'ith the real parameter t e [0, 1]. By Proposition 29.72(a), we obtain the
following results:
(i) Let (K,g) E o.!I. Then, for each t in a sufficiently small neighborhood of
1 = 0, equation (148) has a unique solution u, e Dst8bJe in some neighbor-
hood of Uo.
(ii) The map t ...... 14, is C'XJ.
(iii) The solution u, can be uniquely continued as long as u, remains in Dtt.bl'
i.e., 14, remains strongly stable.
(iv) If u, reaches the boundary of stability OD lt . bl " then bifurcation of t.-. u,
may occur.
Explicitly equation (148) means:
-div Lu,(qr) + L..(q,) = (1 - t)K o + tK on G,
u, = (1 - t)90 + tg on oG,
( 148. )
where q, = (x, ",(x), u;(..'(».
29.19g. An Approximation Method
Differentiation of equation (148) with respect to the real parameter l yields
A'(u,) :' = (K, g) - (Ko,90)'

730
29. Noncocrci\'C Equations and Nonlinear Fredholm Alternatives
and discretization of the derivative du,/dt leads to the following approxima-
tion method:
A'(u"4t)(U(n+IJM - u ll4l ) = L1t(K,g) - (Ko,go» (149)
for fixed At > 0 and n = 0, I,... . Explicitly, equation (149) means
J(r4 n4 ,)u. II + 1 ).41 = J(U.&)U"4f + t(K - Ko) on G,
"(.+1)4' = 14 rt4t + At(g - go) on oG,
where J denotes the Jacobi differential operator (142).
Therefore, in each step n = 0, I, 2...., we have to solve a linear strongly
elliptic system in order to find u..... 1 )41. The convergence oCthis approximation
method as At --+ 0, and the simple physical interpretation of this method, in
terms of elasticity, will be considered in Section 61.16.
(149*)
29.20. A General Bifurcation Theorem for
the Euler Equations and Stability
Along with the variational problem
fG 1.(x, u(x), u'(x))dx - P fG R(u(x)) dx = stationary!,
u = 0 on oG,
where P is a real parameter, we consider the corresponding Euler equation
( 1 SO)
-div Ly.(q) + L..(q) - PR'(u) = 0
.
u = 0 on oG,
where q = (x, u(x), u'(x». Moreover, we also consider the corresponding
Jacobi equation at 14 = 0:
on G,
(151)
J(O, Pcrtt)h = 0 on G,
h = 0 on cG,
(1 52)
where we set
J(u, P) = - div(L"'u,(q)h' + L...,,(q)h) + L.....(q)h' + L".,(q)h - PR"(O)h.
Observe that (152) Collows from (ISI) by linearization with respect to 14 at
u = o. The explicit Corm of equation (151) and (152) follows from (135*) and
(142*), respectively.
We make the Collowing assumptions.
(H I) G is a bounded region in R{\' with aG e CZ.S where N  I and 0 < a < 1.
Let u = (u 1 , . . . , u. v ), M > 1.

29.20. A General Bifurcation Theorem (or the Euler Equations and Stability
731
(H2) The functions L: G x R'" X R NM --. (Ii and R: R M --.(R are C. Suppose
that
L..,(x, 0,0) = 0,
Lu(x, 0, 0) == 0,
R'(O) = 0,
Le., u = 0 is a trivial solution of the Euler equation (151), and suppose
that
R"(0)/J 2 > 0 for all h e R. v - {O}.
(H3) The strong Legendre-Hada,nard condition is satisfied at u = 0, i.e.,
L....(x, 0, O)(d 0 V)2 > 0
for all x e G and all nonzero d e RI\., v E R.".
We set
x = {u e C:Z.( G )M: u = ° on oG},
Y = C«( G )"',
and on the B-space Y, we introduce the scalar product
(fig) = fG fg dx for all /. g e Y.
In terms of elasticity, the function u describes the displacement of an elastic
body, and P describes an outer force. The variational problem (150) cor-
responds to the principle of stationary potential energy, where we have:
fG L dx = elastic potential energy,
p fG R(u)dx = work of the outer force.
We are looking for nontrivial solutions u  0 of the Euler equation (151). This
corresponds to the buckling of beams, rods, and plates for the critical outer
force Perit. The following propositions generalize our results about the buck-
ling of beams in Section 29.13.
Theorem 29.M (Bifurcation). Assume (H 1 )-(H3), and assr4me that, for fixed real
P crit ' the linearized equation (152) has exactly n linearly independent soll4tions
h  0, where n  1.
Then (u. P) = (0, Pcril) is a bifurcation point of the Euler equation (151) in the
space X x IR.
If, in additiolJ, the functions Land R are even with respect to u and u', ,lien
llaere exist "n branches" of nontrivial solutions of the Euler equation (151) in a
neighborhood of tIle point (0, Peril) in the space X x R. This is to be understood
ill the sense of Corollar}' 29.63.
A solution u of the Euler equation (151) is called stable if it corresponds to
a strict local minimum of the original variational problem (150) in the space X.

732
29. Noncoerci\'c Equations and Nonlinear Fredholm Alternatives
"1
I
P
p.;nt
Figure 29.23
CoroUar)' 29.75 (Simple Eigenvalue Peril and Stability). Assume (H I )-(H3), and
clssume lltat ti,e .functions Land Rare anal}'tic ,vith respect 10 tile variables u
alld u'. Moreover, assume that tile linearized eqllatio'J (152) has exact ,>, one
linearl):, independent solrltion de,wted b}' hI' ",here hI i= O i,e.. P crh is si",ple.
Then there exists a neighborhood V of tile poi'Jt (u, P) = (0, Prit) i'l tile space
X x IR such tllat all the nontrivial so/rltions of the Euler equation (151) in V are
git'elJ b}' tile ancll}ftic ('url SI-+(II,P), ",ltere
P = Peril + £1 5 + £2 S2 + O(s-'),
u = sll. + S 2 U 2 + 0(S3),
S -+ 0,
(153)
and s i, a sInal1 real paranleter i'J a neighborllood of s = O. The trivial SOllllions
oj. (151) are gitn by II = 0, P = arbitrar}'. If the bifurcation is supercritical
(Fig. 29.23), i.e., for fixed k = 1, 2, . . . and £2k > 0, "'e 'Jave
P = Peril + t 2A S 21c + O(S21c i 1 
SO,
and if Peril is the sIJlallest eigenvalue of (152), then t/Jere e."Cist positive nllmbers
So and £0 such that the follo,,';ng hold.
(a) Tile bifurcation soll,tion (153) ;S stable for 0 < Isl s so.
(b) The trivial soilltio" rI = 0 is stable for Peril - eo < P < Perle and unstable
.for P Cfil < P < PC'il + Bo.
PROOF OF THEOREM 29,M. Let P = P crit + e, We write problems (ISO) and (1 51)
in the form
1(14) = stationary!,
u e X
and
H(II, £) = 0,
u eX, £ e IR,
respectively. Then the linearized equation (152) corresponds to the equation
H,,(O,O)h = 0,
he X.
The assertion now follows from Theorem 29.K, Corollary 29.63, and Proposi-
tion 29.71 (properties of the Euler operator).

29.21.  Local Multiplicity Theorm
733
In this connection, observe that we may assume that RN(O) = I after a linear
transformation of the coordinates (u 1 ,..., UM), if necessary. This immedi-
ately implies the generic branching condition (H4) from Theorem 29.K, since
HUf(O,O)II£ = - tR-' (0)/1 = - eh. and hence
(hIHur(O,O)h) = -(hlh) #: 0 if II  o.
o
PROOF OF COROLLARY 29.75. The proof proceeds completely analogously to
the corresponding proof for the buckling of beams (Proposition 29.48) by
using Theorems 8.A and 8.E and the eigenvalue criterion from Proposition
29.69.
In this connection. note that the eigenvalue equation
11,,(u. £)/. = Jill.
heX.. pER,
from Theorem 8.E corresponds to the Jacobi equation
J(II.. P)/a = #,h..
hEX, 1J E iii,
",'here P = P': ril + £. Theorem 8.E tells us the sign of the smallest eigenvalue
#'min along the two solution branches (153) and I' = 0, I; = small. This implies
assertions (a) and (b). Note that P.: ril is the smallest eigenvalue of (152) and. as
above, we may assume that RN(O)h = h for all hEX. 0
29.21. A Local Multiplicity Theorem
We want to study the equation
Au = b,
U E U(u o ).
( I S4)
\vhcrc U(llo) denotes an open neighborhood of the point U o in the B-space X.
Our goal is to generalize the situation pictured in Figure 29.24(a), where we
have the following: If b = b o .. b > b o .. and b < b o , then the number of solutions
of equation (154) is equal to one, two, and zero, respectively.
Defmition 29.76. Let C be a subset of a B-spacc Y over D< = iii, 'C, and let r > 1.
Then C is called a c r -manifold of codilnension one in Y iff, for each point b o e C.
b
A
c
N
b o
II
Uo
(a)
T
(b)
Figure 29.24

734
29. Noncocrci\'c Equations and Nonlinear Fredholm Alternati\'eS
there exists a Cr-Cunctionalf: U(b o ) S Y -. (K such thatf'(b o ) =F 0 and there is
some neighborhood V of b o such that
Crt V= {be V:f(b)=O},
i.e., C can be described locally by the equation f(b) = 0 (see Fig. 29.24(b) for
Y = R 2 ).
We assume:
(HI) The operator A: U(uo) S X -+ Y is C t and Fredholm of index zero,
where X and Y are real B-spaces and 2  k S 00.
(H2) Let dim N(A'(uo» = 1 and suppose that the crucial generic branching
condition
A"(u o )h 2 f R(A'(uo)
(155)
is valid for some II e N(A'(uo». Finally, let D'ioa denote the set of singular
points of the operator A.
In Figure 29.24(a), we have A'(uo) = 0, and condition (155) means
A"(u o ) :F- O.
Proposition 29.77. Assume (H 1), (H2). Then there exist a neighborhood V(uo)
of "0 alad a neighborhood W(Auo) of Auo such that the follo\ving hold:
(a) The local set of silJgular values of the operator A,
C = A (D sinl " V(uo»,
;s a C Ic - 1 -manifold of codimension one ill 1':
. .
(b) There exist nonemply open connected sets T and N ,,'itll
W(Au o ) = Cu Tu N,
such fllat the original equation (154) has
(i) e."(actl}, Olle solut;Oll for b E C,
(ii) exact/}' "\10 solutions for beT, and
(iii) no .olutio'l for b e N (Fig. 29.24(b»).
PROOF. Letting
F(t,x) def A(u o + x) - (b o + e) = 0, x e X, t e y,
the assertion is a special case of Theorem 8.F. Condition (155) corresponds to
the generic branching condition of Theorem 8.F:
a. ctg (xT, F:c:c(O, O)x) ¥- 0,
where II = x I. Furthermore, observe that the proof of Theorem 8.F shows
that, for all I; e Y,
;"(0)£ = a8,(0,0)£ = a(xT, (O, 0)£).

29.22. A Global Multiplicity Theorem
735
In our present case. we have (O, 0)8 = - s. Hence 1.'(0) = - axf. This implies
y'(O) #: o.
Therefore, the equation y(t) = 0 in Theorem 8.F describes a Ct-1-manifold of
codimension one in a neighborhood of I: = 0 in 1': 0
29.22. A Global Multiplicity Theorem
We now want to globalize the preceding result. To this end. we consider the
operator equation
Au = b.
ue x.
(156)
(H I) Let A: X -+ Y be a proper Ct.Frcdholm operator of index zero, where X
and Yare real B-spaces and 2 < k < 00.
(H2) The set DSln. of the singular points of the operator A is nonempty. closed,
and connected. Furthermore, A is injective on D'inl.
(H3) If u e DS1D8 then dim N(A'(u» = I and
A N (u)h 2 _ R(A'(u) for some h e N(A'(u»).
In the special case X = Y = R. condition (H3) means that A'(u) = 0 implies
A"(u) #: o.
Theorem 29.N (Ambrosetti and Prodi (1972». ASSfllne (HI)-(H3). Then the
set C = A (D sins ) of the sillgular values of tile operator A is a closed cOlJnected
C" -l.manrold of codimension one ilJ 1':
There e,,=iSllWO nonenaply open connecled subsets T and N of Y with
Y=CuTuN,
.'ch tllat the original equatiola (156) has
(i) e.'tCacll}t one solution for b e C,
(ii) exacll}t ""'0 solutions for b e 1: and
(iii) no solution for b eN.
PROOF. According to Theorem 29.E and Proposition 29.77. it is sufficient to
show that the set Y - C consists precisely of t\\'O components.
Since the set D sina is connected, so is C. Moreover, since D Sinl is closed and
the operator A is proper. the set C is also closed. Let B be a componenl of the
set Y - C, i.e., B is a maximally connected open subset of Y - C with respect
to the induced topology on Y - C. Since Y - C is open in Y: so is B. The set
aB is not empty. Otherwise, we \\'ould have B = Y.
Let b e oB. Then b e C. The set aB is closed. By Proposition 29.77, there
exists a neighborhood Web) of the point b such that Web) tl C c oB. Hence
the set iJB is open alad closed in C. Since C is connected, we obtain the ke}' result
oB = C.

736
29. Noncoerci\'c Equations and Nonlinear Fredholm Alternatives
Again by Proposition 29.77, the set Y - C has locally two components.
Consequently, Y - C has globally two components. 0
Applications of this theorem to semilincar elliptic differential equations can
be found in Problem 29.9.
PROBLEMS
29.1. Coincidence degree in H-spaces. In order to explain the simple basic idea of
the general coincidence degree in Problem 29.2, we first consider a special
case. \Ve investigate the equation
Bu = J\lu.
ueG..
( 1 57)
where G is a nonempty bounded open set in the H-spacc X. We make the
folloy,'ing assumptions:
(i) The linear continuous operator B: X -+ X is Fredholm of index 7.ero,
i.e., the range R(B) is closed and
dim N(B) = dim R(B)l < 00.
Here, R(B).l. denotes the orthogonal complement to R(B).
(ii) The operator ,'1: X -. X is compact.
(iii) \Ve fix an orientation in both N(B) and R(B)1 and choose a linear
bijective orientation-preserving operator
J: J\J(B) ... R(B).J..
Let Q: X -+ N(B) be the onhogonaJ projector onto the null space N(B)
of B. Now" our key definition reads as follows:
Su - Bu + JQu
for all u EX.
29.1 a. Sho\\' that S: X ..... X is bijective.
Solution: See the proof of Proposition 29.3.
29.1 b. Shoy,. that the original equation (157) is equivalent to the equation
u = S-I j\fu + S-IJQU. (157*)
Solution: Equation (157) is equivalent to Su = j\fu + JQu.
29.lc. Sho\\' that the operator
C = S-IM + S-lJQ
is compact on X.
Solution: The operator S-I: X ... X is continuous according to the open
mapping theorem A J (36). The operator M: X .-. X is compact by hypothesis.
Finally.. the operator Q is compact since dim Q(X) = dim N(B) < x). Hence
(. is compacL
29.ld. Definition or the coincidence degree. Suppose that
Bu  Mu on aGe
Then Cu  u on aG. Thus, the Leray-Schauder degree deg(l - C" G) is well

Problems
737
defined, and we set
deg(B, JW; G) = deg(l - C, G).
29.1c.* Show that deg(B, M; G) docs not depend on the choice of J, i.e.. deg(B. .W; G)
depends only on the orientation of J\J(B) and R(B) t. Moreo\'er. show that if
we change the orientation of either N(B) or R(B)!, then deg(B, .W; G) changes
its sign.
Hint: This is a special case of Problem 29.2e.
29.2. The general co;nt.'idence degree of JW a'hin (1972). We now study the equation
Bu - M u,
u e G,", D(B).
( 158)
We make the follo\\'ing assumptions:
(H I) X and Y arc B-spaccs over f( = R. C.
(H2) G is a nonempty bounded open set in X.
(li3) The linear operator B: D(B) s; X  Y is Fredholm of index zero, i.e.,
R(B) is closed and
dim J\J(B) = codim R(B) < 00.
(159)
(H4) The operator M: G  X -. Y is B-compact. By definition, this means
that the operator M: G  Y is continuous and bounded and the opera-
tor K P.W: G  X below is compact.
As we shall see below, this notion depends only on M and B. For
example, j\-t is B-compact if one of the following two conditions is valid:
(i) .f is compact
(ii) .Id is continuous and bounded and K: R(B) -. X is compact.
We call the pair {B, M} adnlissible on G iff(HI) through (H4) hold.
29.2a. Projections. Let
Q: X -+ N(B).
P: y..... R(B
be projection operators onto N(B) and R(B respectively.
We set Q.1. .. 1 - Q and pl. = I - P as well as
N(B).1. = QJ.(X
R(B).1. = p.1( Y).
Then we obtain the topological direct sums
x = N(B)G) !\'(B).l,
Y = R(B) EB R(B)-.
The operator
B: [\'(B)1 () D(B)  R(B)
is bijective. Let
K: R(B)..... N(B)J. ra D(B)
be the corresponding inverse operator. i.e., BKb = b on R(B).
We fix an orientation in both N(B) and the factor space Yj'R(B). The
canonical mapping
j(u) = u + R(B)
is a linear bijective map from R(B).l onto YIR(B Let
J: N (B) ..... R(B)l

738
29. Noncoerci...c Equations and Nonlinear FredhoJm Alternatives
be a linear bijective map so that j 0 J: J'I(B) -. Y{R(B) is orientation.
preserving.
29.2b. Show that thc B-compactncss of f in (H4) depends only on ,\-, and B.
Hint: One has to show that the compactness of KPJ\1: G -+ X docs not
depend on the choice of the projection operators P and Q. Cf. Gaines and
Mawhin (1917). p. 13.
29.2c. Our key definition reads as follows:
Su = 8u + JQu
for all 14 e D( B).
As in the proof of Proposition 29.3 it follows that S: D(B) -+ Y is bijccti\'c and
S-lb=Kb onR(B).
( 160)
The originaJ equation Bu IiiiiI Mu, u E G" D(B), is equivalent to the basic
equation
(E)
u = S-I Mu + S-I JQu.
Show that the operator
C = S.-I j'd + S-I JQ
is compact on G.
Solution: It follows from (160) that
C = KPM + S-I PJ.M + S-IJQ.
By (H4). the operator K P,\-I is compact on G. The projection operators p:.
and Q have finite-dimensional range, and the linear operator S-I is continu-
ous on tinite.dimensional spaces.
29.2d. Definition (if the coincidence degree. Let {B, \-I} be admissible on G. Then thc
Leray-Schauder degree deg(1 - C, G) is well defined and we set
deg(B, M; G) = dcg(1 - C, G).
If G = 0. then let deg(B, M: G) = O.
29.2e.- Sho\\' that deg(B, M: G) is independent of P. Q. and J. i.e., the coincidence
degree dcg(B J M; G) depends only on the orientation chosen in both N (B) and
Y/R(B). If we change the orientation of either N(B) and YfR(B), then the
coincidence degree changes its sign.
Hint: Use the representation
C = KPM + J-aplJ\1 + Q
given in Proposition 29.3, and use the topological in\'ariance ("I') of the
Leray-Schauder degree in Section 13.7. Cf. Gaines and Ma\\'hin (1977), p. 19.
29.3. Properties of the coincidence degree.
29.3a. Existen,'e principle. Show that if deg(B, J"'; G) :I: 0, then the equation Bu =
JWu, u e G r. D(B), has a solution.
Solution: By hypothesis, deg(l - C. G) :I: O. Hence the equation Crl = 14"
U e G, has a solution. This equation is equivalent to Bu = Mu, U E G r. D(B).

Problem8
739
29.3b. Additlvit)' propert}'. Let
.
G = U G i .
t-l
where {G f } is a family of pairwise disjoint bounded open sets. Let {B. M} be
admissible on G and let
Bu :;: JWU on cG i
for all i.
Show that
.
deg(B, M; G) = L deg(B, JW; G,).
i=1
Solution: This follows from the corresponding property of the Lcray-
Schauder degree.
29.3c. Homotop)' invar;ance. Suppose that the map
II: G x [0, t] -4 X
has the property that {8, H(.,,t)} is admissible on G for all A e [0, 1] and the
operator H is B-compact. i.e.. KPH: G x [0. I] -+ X is compact. Show that
dcg(B. H ( . , ,t): G) = const
for all A E [0, 1].
Solution: This is a consequence of the homotopy invariance of the Leray-
Schauder degree.
29.3d. Continuation principle. Suppose that:
(i) {B. M} is admissible on G.
(ii) Bu  ).,"Iu for all (u,).) E oG x ]0, I].
(iii) deg(J- ' pJ. ,\1, G n N(B» #: O.
Show that the equation Bu = Mu. u e G ("\ D(8). has a solution.
Hint: cr. Gaines and Mawhin (1977). pp. 29 and 40.
29.3e. Reduct;on principle. Let {8, M} admissible with R(.W) 5 R(8).l.. Sho\\' that
Ideg(B, M; G)I = Ideg(J- 1 .\1, G ('\ N(8)I.
Solution: We have
C = KP,'d + J- 1 pJ. M + Q = )-1 J\I + Q,
Hence I - C = -J- 1 M on N(B). By the reduction principle (R) of the
Lcray-Schauder degree in Section 13.6. we obtain that
deg(B, M; G) = deg(l - C. G) = dcg(l - C. G r. N(B»
= dcg( -J- 1 M. G r. N(B» = (- Ifim"'CB} deg(J- 1 M. G r. N(B)).
29.4. Application to periodic solutions of differential eqlUJtions. Let 0 < T <  be
fixed. We consider the problem
U'(I) ;; f(t. U(f») on [0, T],
u(O) Iiia u(T),
(161)
i.e., we seek periodic sol u tions u: [0, T] -.. AN.

740
29. Noncoerci\.c Equations and Nonlinear Fredholm Alternatives
29.4a. Operator equation. Show that this problem can be reduced to the equation
Bu = .Wu,
ue G.
( 162)
Solution: We set
Bu = u'.
(Mu)(t) = f(l. U(I))
and
x = y = C([O, T], RN
O(B) = {u e C1([O, T], R"'): u(O) .. u(T)}.
Then
.1\1(8) ;:: {u EX: " = const}.
R(B) = {.'E Y: f: }'(I)dl ,. o}.
The projection operators Q: X  N(B) and P: Y -.. R(B) can be defined by
Qu = u(O), (PY)(I) = yet) - r- I f: y(l)dl.
Then . (B).1. = (I - Q)(X) and R(B)J. = (I - P)( Y). i.e.,
N(B)J. ;;:: {II E X: 11(0) = O}.
R(B)J. = {}' e Y: }' = const}.
The operator B: lv(B)l r. D(B) -+ R(B) is bijective. and the inverse operator
K: R(B) --. J\J(B)l ,... D(8) is given by
(Ky)(l) = L }'(s)ds.
The operator K is compact, i.e., M is B-compact. The bijccti\'c linear operator
J: J'J(B) -. R(B)J can be dcfined by
Ju = u on N(B).
29.4b. C()nlpute the coincidence degree of (162).
Solution: B)' Problem 29.2, equation (162) is cqui\'alent to
u = Cu,
ue G.
( 162- )
with
C = KPM + J-I(/ - P)M + Q.
Hence
(CU)(I) = L (/(s,u(s» - a)ds + a + ufO)
with a = T- 1 J 1(5. u(s» ds and
deg(B, M; G) = dcg(l - C. G),
29.4c. An importanl propert}. of the Brou,,'er degree for potemial operalor.... Suppose
that:

Problcms
741
(H) The function Y: R N -+ R is C. and \\'cakly coercive. i.e., V(x) -+ + 00 as
Ixl ..... oc. Furthermore. there is an r > 0 such that V'(x) :;: ° for all x:
Ixl  r.
Let U(O.r) = {x E A"': I.I < r}. Sho\\' that
deg( V'.ll(O. r)) = 1.
Solution: This is a special case of Problem 14.4<1.
29.4<1. Gradient s }'stems. We consider (161) \\'ith 1(1, u) = - V'(u), i.e., \\'e consider the
gradient systcm
Il = - V'(u) on [0. T],
u(O) = 1'( T).
Suppose that condition (H) holds and set G :: {u e X: lIuD < r}. Sho\\' that
(163)
Ideg(B, j"'; G)I m; deg(V', U(O, r)) s: I.
( 164)
This relation is the ke)' to the general existence theorem in Problem 29.4e
bc I 0\\' .
Moreover sho\\' that the function u is a solution of (163) iff u = const = c
and V'(,") = O. Since deg(V', U(O,r)) = 1 by Problem 29.4c, there exists at least
one such solution of (163) with c e U(O, r).
Solution: We set pl. = I - P and use the homotopy
u' = -i.V'(u) - (I - i.)T-1 J: V'(u(t»dt,
u(O) == u( 1),
( 165)
where 0  ).  1. This equation corresponds to
Bu = )'Mu + (I - ),)Pl.Mu,
ue G.
(t 66)
where we use the notation of Problem 29.4a.
(I) \Vc sho\\' that this is a homotopy. Let u be a solution of( 166). i.e., il ull < r.
Multiplication of(165) with u' and subsequent integration )'ield
J: (u'(I)lu'(I»dl = O.
In this connection, note that V(u(t)' == < V'(u(t»lu'(t» and u(O) = u(T).
Thus, u = const. and (165) yields u'(O) = Oand hence V'(u(O)) == O. By (H),
114(0)1 < r. That means u e G.
(II) The homotopy invariance of the coincidence degree yields that, for;' - I
and A = 0,
deg(B. M; G) = deg(B, P l j\f, G),
and it follo\\'s from the reduction property (Problem 29.3e) that
Ideg(B, p.l M. G)I =- Ideg(J- 1 pJ. M, G" N(B))I.
On N(B), the operator J -I p.l. M is identical to V': N(B) .-. N(B). Because
of l\'(B) = R N , we obtain
dcg(J-. pl. M. G r. N(B» = deg(V', U(O, r»).
By Problem 29.4<:. deg(V', U(O,r)) = 1.

742
29. Noncoerci\'e Equations and Nonlinear Fredholm Alternatives
29.4e. A general e:(istence theorem. Suppose that:
(i) The function f: [0, T] )( R'v ... R.... is continuous.
(ii) There exists a C1.function V: (RN  R with V(_) ... + 00 as I..I-+ 00, and
there exists a function g e C[O, T] such that
(V'(X)l!(I, x» < g(t) for all (x. t) E R N X [0, T].
(iii) There exist a number r > 0 and a CI.function W: tR N - V (0, r) --+ R
such that
(V'(x)IW'(x) > 0
for all x with Ix I  r, and
J: (W'(u(t»lf(t.u(l)))dt  0
for all C'.functions u: [0. .J-] ..... (RN with u(O) = u(T) and IU(I)I  r on
[0, T].
Shoy" that the original problem (161) has a solution.
Hint: Use the homotopy
u' = (1 - ).)/(1. u) - ,t V'(u) on [0. T).
14(0) = u(T).
( 167)
Then the homotopy invariance of the coincidence degree and (164) )'ield
Ideg(B.MG)1 = 1.
and the assertion follows from the existence principle of the coincidence
degree.
In order to show that (167) is a homotopy, one needs a priori estimates
b)' means of the function W. Cf. Mawhin (1979, L). p. 64.
29.4f. A special case. Let /: (R  R be a monotone increasing continuous function.
Show that the problem
u' = f(u) on [0, T].
u(O) = u( T).
has a C1.solution iff there exists a function v e C[O. T] with
f: f(v(x»dx = O.
Solution: Use Problem 29.4e with
V(x) = f:' (1 + yl/2r l dy.
W(x) = J '"' }' -112 dy.
,2
Cf. Mawhin (1979). p. 66.
29.S. COlu/iliol' (S) and linear f.redholm operators of index zero.
Let B: X ... X. be a continuous linear operator satisfying condition (S) on the
real renexive B-space X.

Problems
743
Sho\\' that B is a Fredholm operator of index l.ero.
Solution:
(I) We shoy,. that R(B) is closed. Since B is linear it is sufficient to prove
that B(.\1) is closed for all closed bounded subsets M of X (cf. Dunford
and Sch\\'artz (1958, M). Vol. It Theorem VI, 6.5).
Let (II.) be a sequence in the closed bounded set J\-I and let
Bu" -+ V
as n -t 00.
Since X is reflexive, there is a subsequence, again denoted by (u. such
that
II"  U
as n  00.
Thus, (Bu", II,,)  (v, u) as n  00. By Figure 27.1. (S) implies (5)0'
Consequently,
U" .... u
as n -+ 00.
Hence u e M and Bu = v" i.e., v e B(M).
(II) We sho\\' that dim J\J(B) < 00. The set J\J(B) =: {u E X: Bu m O} is a
closed linear subspacc of X. Set
M = {u e N(B): 111411 < I}.
Letting v = 0, we obtain from (I) that each sequence in .W has
a convergent subsequence, i.e., M is compact. By Theorem 21.C.
dim N(B) < aJ.
(III) Let n = dim N(B) and n* = dim N(B). Suppose first that II > 1. We
show that nit  n.
Otherwise, n* > n. Let {u.,..., II,,} be a basis in N(B and let uT,...,
u: be linearly independent elements in N(B*). Note that B* maps X
into X* since X.. = X. Hence uf EX. By AI (S I), there exist elements
Vi' vf e X* such that
(vr.Uj)x = (Vj,uj)x = 'j' ;.j = (,..., n.
As in Section 29.1, we construct the operator
"
Su = Bu + L (V:. II) VA.
1=1
( 168)
(III-I) We show that SII = 0 implies U = O. Indeed, Su = 0 implies (Su, u) ;:;
O. Since 8* II = 0
. t
<Bu,u> - (u,B.uf> - o.
Applying u to (168) we get < v, u) = 0 for all ;. Hence Su = BII = O.
i.c.. II e span{u l ..... u.}. This yields u = O. since (vr,II J > = 'J'
(111-2) We show that R(S) is closed. The linear continuous operator S: X -+
X. is a strongly continuous perturbation of the (S).operator B. By
Figure 27.1. the operator S satisfies condition (S). Noy,., the same
argument as in (I) above shows that R(S) is closed.
(111-3) By (III-I), the operator S: X  R(B) is bijective. By the open mapping
theorem AI (36), the operator S-I : R(S) -+ X is linear and continuous.
(111-4) Let S,u = Su - lb. For all u with lIuD m R and sufficiently large Rand

744
29. Noncocrci..-e Equations and Nonlinear Fredholm Alternati\'cs
all , E [0, 1]. y,'e obtain
IIS,ull  115-1 II-I lIull - IIbl! > o.
By Theorem 29.8, R(S) = X*.
(111-5) Because of n* > n, there exists a u. E N(B-) with u. :f; 0 and
(v., u*) = 0,
i = I,..., n.
By the Hahn- Banach theorem. there exists a ho e X. y,.ith (b o , u. ),y ..
lIu.lI. Since R(S) = X., there is a Uo with Suo = b o . Hence
!lu.:1 = (Suo, u.) = (Buo, u. > = (uo. B*u.) = O.
This contradicts u. "I: o.
(IV) Let n = O. i.e., N(B) = {O}. Letting S = B and using the same argument
as in (III). we again obtain that n* S n.
(V) We sho\\' that n  n.. Since X is renexive, (B*). = B. The definition
of (S) in Section 27.1 shows that B. satisfies (5) if B has this property.
Now.. replace B by B* and 8* by (8*)* = 8 in (III) and (IV).
(VI) It follows from (III) through (V) that n = n*. i.e.. dim J\j'(8) =
dim I\'(B* 
29.6. * 1.he singular values of functionals. Let F: X  R be a C'-functional on the real,
separable, renexi\'e B-space X \\'ith k > 2 and k > dim N(F(u)) for all u E X.
Suppose that F': X  X. is a Fredholm operator.
Show that the set of singular values of F has zero Lebesgue measure in R.
Hint: cr. Berger (1977, M p. 127. Recall that b is a singular value of F iff
there is a u E X such that F(u) -= band F'(u) iiiiI o.
29.7. A bifurcation theorem in R N (if variali()nall)'pe.
29.7a. Let N  1. We consider the equation
F,,(u, j..) = O.
u E R. v , ;. E Ii,
( 169)
where
F(u.. A) = !Alul l + r(u),
and the function r: U(O) c R'" -t R is C 2 on a neighborhood of u = 0 with
r(u) = o(lul2 u -t O. Show that (0,0) is a bifurcation point of equation (169),
Solution: By the Taylor theorem (Theorem 4.A), it follows that r'(O) = O.
r"(O) = 0, and hence r'(u) = o(lul}, u -+ O. The two problems
r(u) = min!,.
r(u) = max!.
(ulu) = p.
(ulu) = p,
have two different solutions u. and U2 for each sufficiently small p > O. Note
that r(.) cannot be constant on small spheres.. since r(u) = o(luI2 u -4 o.
By the well-kno\\'n classical Lagrange multiplier rule (see Section 43.10),
there exists a real number A. i such that r'(u i ) ;;; - ).;u." and hence
I = _ (r'(u;)lu,) _ 0
1 Ui 1 2
as p..... O.

Problems
745
From f(u.).) = i + r'(u) it follows that
f(Ui' Ai) = 0,
Obviously" Ui -t ° as p ..... O.
29.7b.* Proof of (iii) in Proposition 29.25(H).lfr(.) docs not depend on the parameter
A, then the proof follows from Problem 29.7a by using a new scalar product
on X = (R" induced by a( '. ').
If r( · ) depends on i then the sophisticated proof is based on a detailed
study of dynamical systems by using arguments of the Ljustemik-Schnirelman
theory (ef. Part III) and the theory of isolating blocks of Conle)'.
Hint: Use the dynamical system
; = 1. 2.
U'(t) = - F..(u(t).)
and obser\'e that U'(lo) = 0 implies F.(U(lo),i) = O. Cf Rabinowitz (1977) and
Chow and Hale (1982. M). p. 159.
29.8. A t:ariant of the implicit function theorem (blowing-up technique). We consider
the equation
i.B(t, v) = C(t', i.),
v EX, i. E K,
( 170)
in a neighborhood of the point (0,0). The prototype for (170) is the real
equation ).v 2 = g(;')t3( 1 + O(t)) with the solution  = v in the special CWdSC
;.v 2 =- v). Suppose that:
(i) B: X x X  fR is bilinear and bounded on the B-space X over II( -  C"
and I B(t:, v)1 > c!i 1'11 2 for all veX and fixed c > O.
(ii) C: U(O,O)  X x R -t R is C* in a neighborhood of (0" 0), where 1 < k <
:t). Moreover, as (v,).)  (0,0), we have
C(t', l) = o( II vII 2 )
and
C).<v,;") = o( I: v 1 2 ).
Show that, in a ncighborhood of the point (0,0), equation (170) has a
unique solution curve ). = i.h') through the point (0,0). This cun'c is con-
tinuous on a neighborhood of v = 0 and C away from v = 0.
Proof. Set
l _ C(v, ,t)
F(v, 1) = B(v, v)
).
if t' -:F 0,
if v = 0,
and write equation (170) in the form
F(v, )..) = O.
Then F and F). are continuous in a neighborhood of (0,0) and F.l(O,O) = I.
The assertion no\\' follows from the implicit function theorem (Theorem 4.B
29.9.. A multiplicity theorem/or semilinear equations. We want to study the bound-
ary value problem
- u = F(u) + / on G,
u = 0 on (JG.
(171)

746
29. Noncoerci".e Equations and Nonlinear Fredholm Alternati\'t$
F+ f I
(c)
(a)
(b)
Figure 29.25
To this end.. we consider the Jinear eigenvalue problem
-Au = pu on G,
u = 0 on cG
with the eigen\'alues 0 < III < JJ2 S ... . We set
X = {u e C 2 . I (G): u = 0 on oG}.
( 172)
Y = C1(G),
and we assume:
(H 1) G is a bounded region in R'" with iJG e ClZJ and M > I. 0 <  < I.
(H2) The C).function F: IR -+ (R is strictly monotone increasing and convex
(Fig. 29.25(a». More precisely. let F-V(O) > 0 and
r(u) > 0 and r(u)  0 for all u e R,
o < lim F'(u) < 1'1 < lim F'(u) < JJ2.
.-- u-+
Show that there exists a closed connected CI-manifold C of codimension I
in Y such that Y - C consists of exactly two connected components T. N. and
equation (171) has
(i) one solution U E X for fee,
(ii) two solutions u e X for f E T. and
(iii) no solution for feN.
Hint: Use Theorem 29.N. Cf. Ambrosetti and Prodi (1972 Berger and
Podolak (1975), and Nirenberg (1914, L) p. 117. If we consider the real
equation
(E)
-.6u  F(u) + f.
u e R.
with -  > 0 and fER, then Figure 29.25 gives an intuitive interpretation of
the result above. Note that the intersection points in Figure 29.25 correspond
to the solutions of (E).
More general results can be found in the survey articles by Ambrosetti
(1983) and Ruf(1986).
29.10.* Resonance at the first eigelltlau» of the linearized problem. We consider the
semilinear boundary value problem
- Au(x) - a(x, u(x»)u(.) - f(., u(x) on G.
( 173)
u = 0 on oG,

Problems
747
along with the linearized eigenvalue problem
- u - pu = 0 on G,
u = 0 on oG.
Let G be a bounded region in R'" with oG e C, N > 1, and Jet the functions a,
f: G x R -+ Ii be C«J. Moreover, let PI be the smallest eigenvalue oC(174) with
the corresponding eigenfunction U l' Prove the Collowing.
(a) Nonresonce case. If
( 174)
a(x, u) < JJ I for all (x, u) E G x IR,
and if f is bounded on G x R, then the original problem (173) has a
solution.
(b) Resonance case. If
a(x. u) = PI for all (x, u) e G x Rt
and if f,,(x. u)  0 for all (x. u) e G x R, then (173) has a solution iff there
exists a function t 1 e CtX>(G) such that v = 0 on oG and
L f(x,v(x»ua(x)dx = O. (175)
In the case where the function f docs not depend on 14, condition (175)
represents the well-known classical solvability condition for (173).
Hint: Cf. Kazdan and Warner (1975). The proof is based on the method of
subsolutions and supersoJutions from Chapter 7.
Further important material about semilinear elliptic differential equations
can be found in the problems section of Chapter 49 (variational methods).
Cf. also Problem 29.14.
29.11. Stable homotop)' (generalized mapping degree) and the solt'abilit), of nonlinear
operator equations. In the following we will use tools from Section 16.8. Let N.
J\f > I.
29.11a. Essential maps. We consider the equation
F(x) = 0,
x e B ri ,
( 176)
where B N denotes the closed unit ball in IR N . As usual let SN-1 = asH. The
continuous map
f: i) B. ti ..... R M
( 177)
is called essential with respect to B''i iff f(x) #: 0 on 08'" and equation (176)
has a solution for each continuous map
F: 8'" -. R'" with F: / on cB. ti .
From Chapter 16 we obtain the following results:
(i) For /\' m M Q 1, the map / in (177) is essential with respect to 8". =
[ - 1, 1] iff
f(l)f( -I)  O.
(ii) For N = M, the continuous map / in (177) is essential with respect to B N

748
29. Noncoer1\'e Equations and Nonlinear Fredholm Alternatives
iff f(x)  0 on vB N and
deg(f, int B N ) :I- O.
(iii) For f > J\', each continuous map (177) is nonessential with respect to
8"'.
The following methods can be applied if M  tv..
29.11 b. J\Jullhomotopic' maps. The continuous map
9: S.Y -1  S." -1
is called lJullhomotopic (or homotopically trivial) iff it can be deformed into a
constant map, i.e., there is a continuous map
H: S. - t X [0, I] -+ S'w 1
such that II(x,O) = g(x) and II(x, I) = const for all . e SN -I.
According to Example 16,24, the continuous map f: cB N -+ RAt - {O} is
essential with respect to 8 N iff the normalized map
L: oB N  oB."
1/1
is not nullhomotopic.
29.1 Ie. l\'ontr;vial stable homotopy.l.-et /\'  .\1  I. We consider the continuous map
g: SN-l -+ SII-I
( 178)
along with the Kth suspension
SKg: SN-I..a: -. S",-I+«.
( 179)
The suspension operator S has been introduced in Section 16.8. An impor-
tant topological result tells us the following.
For K  N - J\I. tI,e map SK 9 in (179) is not nu/llJomotopic iff S«., 9 is not
nul/homotopic.
Let 8;" = {x E R''': Ixl  r}. and let g(x) = f(x)/lf(x)l. The continuous map
f: B.  R'" (180)
is said to have stable homotopy iff f(,v;) #: 0 on iJB and all the suspensions SKg
in ( 179) with K :> I are not nullhomotopic.
(i) For JV := M, the continuous map f in (180) has stable homotopy iff it is
essential \\'ith respect to B:, i.e., f(x)  0 on 08;" and
deg(/, int B:) #: O.
In the case N = J'" = I, this means f(I)/( - t) :1= O.
(ii) For N > .M, the continuous map J' in (180) has stable homotopy iff
f(x)  0 on oBf and all the suspensions S'"9 in (179) with I < K <
l\' - .W are not null homotopic.
29.lld. TIle ke) result. Let I < J* < d < .. We set
f .. (  ", ) - { h(I."'. )
I I."' N -
i
if I < i < d*.
if ;  d + I,

Problems
749
where the map
f: Jrf  R'"
is continuous with !(}') -:# 0 on cB". Show that the map
f.: cB,tJ -. RN-"....
(181)
is essential ".jth respect to B,tJ if the map f has stable homotopy.
Solution: It is not difficult to show that the normalized map
II : 5"-1 _ SN-'U.-I
is homotopic to the (N - d)-fold suspension of the map
1.. -I _ 5""-1
If I. ·
and hence F/IFI is not nullhomotopic. since f has stable homotopy.
By Problem 29.11 b, the map F is essential.
29.11e.. The abstract main IheorenJ on semilinear operator equations \\'ith bowJded
IIonli"earities. The equation
Lu = Fu,
ue X,
( 182)
has a solution in the case where the following conditions are satisfied:
(i) I..inear operator. The linear continuous map L: X  Y is a Fredholm
operator of index ind L  0, where X and Yare real B.spaces. We choose
fixed topological direct sums
x = N(L) (f3 J'l(L)J
and
Y = R(L) (!) R(L)l.
along with the corresponding linear projection operator P: Y ..... R(L)J..
(ii) NtJlJlinear perturbation. The map fo-: X ... Y is conapacr. There arc positive
constants rand p such that
II (I - P)Fu II  p
for all u eX.
( 183)
and
F(v + 'v) _ R(/4)
( 184)
for all ve .1\1(1.,), ". e N(L)J. with IIvll > r and II wll S : Lclilp. where Lo
denotes the restriction of L to N (L)J..
(iii) Stable hOmOIOp}'. Let B, = (u e N(L): lIuli  r}. The finite-dimensional
map
PF: H,  N(L) .... R(L)J.
has stable homotopy.
Remark. Condition ( 183) is satisfied in the case where F is bounded, i.e.,
sup IIFul < 00.
IIC X
If ind L = 0 and dim N(L) > O. then condition (iii) holds true iff
deg(PF, int 8,) * O.

750
29. Noncoercive Equations and Nonlinear Fredholm Alternatives
If ind L > 0, then it is not possible to formulate condition (iii) in terms of
the mapping degree, since the map PF lowers dimension.
Hint: cr. Cronin (1973), Nirenberg (1974. L), p. 134, Berger and Podolak
(1974), (1975), and Berger (1977, M), p. 279.
29.11 f.. Application to general semilinear ellip';,- boundar)' t'tllue problems ,,'ith nonega..
l;t'e index (the generalized Landesnwn-Lazer theorem). We consider the
boundary value problem
Lu(x) =- f(x. u(x)) on G.
Bu = 0 on oG.
( 185)
where
Lu = L DGlY'u
,crt s 1-
and Bu - (B 1 u,..., BKu) with Bill = LIM < 2..1\.,O"U.
Show that problem (185) has a classical solution II in the case where the
following hold.
(HI) G is a bounded region in R'\' with oG e C. where N, m  I. All the
functions a«, 1\.,: G ... R are COX).
(H2) The linear differential operator L is elliptic. i.e.,
 a(x)l)G  0 for all x e G. D e (RN - {O},
1d! - 2.
where D = (D. 1 ..., D.\,) and D 1 = D:- D;2 "' D.'''.
(H3) The boundary operator B satisfies the algebraic complementing condi-
tion. According to Problem 6.8, large classes of boundary conditions
satisfy this condition. For example, we can choose the boundary condi-
tion Bu = 0 in the form D'u = 0 on aG for all y: h'l S m - 1.
(H4) Let 9 D o. The only solution of
Lu - g on G,
Bu = 0 on cG.
( t 86)
which vanishes on a set of positive measure, is u  o.
(H S) Let {u I' . . . , U4} be a basis or the solution set of (186) \\'ith 9 = 0, and let
{II"..., u".} be a cobasis. i.e., for given 9 e C 2J (G), problem (186) has a
solution u iff
L g(x)ut(x)dx = 0
Suppose that I < d. < d.
(H6) The function f: G x R... R is C<7;, and
for i = I, II . , d*.
If(x, u)1 < const
for all x e G, u E R.
The limit
hex. t» = lim I(x. rv)
r-+C%)
exists uniformly for all x e G and v e R with Ivl = 1.

Problems
751
(H7) The ke}. condition. We set F .. (Fa,..., F".) with
F;(c) = f ut(x)h ( x. t CJUJ(X» ) dx
JG J-t
and c = (c I . . . . . C4). where cJ is real for all j. Suppose that the map
F: IJ4  
has stable homotopy.
In particular, if d :I: d. t then (H7) holds in the case \\'here
deg(F, int ) :F o.
If d = d. = 1. then (H7) is equivalent to
F 1 (1) F. ( - I) < o.
Hint: cr. Nirenbcrg(1970 (1974. L p. 140.
A variant or this theorem can be round in Schechter (1973) by replacing
condition (H7) with inequalities.
29.12. A general model for beams and its stability. We consider a beam oflcngth 1 as
pictured in Figure 29.26. In an idealized form, let the deformed beam be
described by the curve
 m 2 + a( tX).
, = C(2).
o  « S I,
(181)
\\'here t 'It , are Cartesian coordinate and u = (a, c) is the displacement
vector in the (, C)-plane. More precisely, the undeformed beam is given by
the set
((,'1,') E R3: 0   < I, (".{)e Q()},
where Q() denotes the cross setion of the beam. To simplify our considera-
tions, let us assume that the cross section Q is constant, i.e.. it is independent

beam
/

p
'1
(a) P < P.:rn

(. )
p
a
(b) P > Pral
Figure 29.26

752
29. Noncoercive Equarions and Nonlinear Fredholm Alternatives
of';. Variable cross sections will be studied in Section 64.7. The number P > 0
is the strength of an outer force acting in the direction of the negative -axis.
We want to motivate the following varwliollal principle for the functions a. c:
dt' r I A 2 B 1 .
Epee = Jo I k + 2 (t' - I) d + Pa(/) = mID!.
a(O) = c(O) = 0,
c(/) = o.
( 188)
In this connection"
« I + a')c" - a" C')2
k=
(I .t- a' 1 + c' z )-'
is the CrlrlIUre of (187) and
r' = J I + a'2 + C'2
is the derivative of the arclength of (181). The boundary condition corre-
sponds to Figure 29.26.
J\lotivation. According to Section 61.6. the potential cnergy Epot of an
clastic body under the displacement u is given by
Epoc(u) = U(u) - ut(u)t
where 1.1 is the elastic potential cnergy and Woua(u) is the work of the outer
forces with respect to the displacement u. In our special case. y,'c obtain that
Wout(u)  - Pa(/
U :: (a. c),
since work = force times displacement, and the force acts in direction of thc
negative  -axis.
\Ve now assume that the elastic potential encrgy U depends on
(i) the curvature k of the beam, and on
(ii) the length contraction of the beam.
Ad(i). Suppose that U has the form
u... E f(k)dk.
For small curvature k, Taylor expansion yields
f(k) !: 1(0) + f'(O)k + !f N (O)k 2 + ... .
It is natural to assume that k = 0 implies f(k) = 0 and hence 1(0) = O.
Moreo\'cr. we assume that f does not depend on the sign or the curvaturc.
This yields the second-order approximation
A
f(k) = I k2 .
where A depends on the material and the cross section. In Section 64.7 we
shall motivate that
A = EJ. J = fa {ld"d'.
whcre E is the so-called clasticity module related to Hooke"s law (189) below.

Problems
753
Ad(ii). We consider two points (. 0,0) and ( + l1" 0, 0) of the undeformed
beam. The relative change of length of this clement of the beam is equal
to
I = (6t - A/6,
\\'here r denotes the arclength of the deformed bc:am. By Hooke's law, in
Section 60.1. the corresponding tension is given by
a =- Ei'.
( 189)
We now consider a volume element l1V = m(Q) or the undcrformcd beam,
where m(Q) denotes the measure of the constant cross section Q. According to
Section 61.7, the clastic potential energy
!ayV
corresponds to the strain j'. This leads to the tcrm (  )(r' - 1)2 d in (188)
with
B = m(Q)E.
Study the stability of the trivial solution a() = 0, c(,) = 0, and determine
the buckling force PICric (Fig. 29.26).
Hint: Use similar arguments as in Section 29.13 to obtain that
( An 2 )
P erit = min 1 2 -, B ·
If the length of the beam 1 is sufficiently large, i.e.. An 2 /1 2 < 8, then we obtain
the classical Euler buckling force Pcr't = A Jt2 /1 2 . Note that our model (188) is
more general than (97 Cf. Klotzler (1971, M), p. 147.
29.13.. An existence theorem for semilinear operator equations in H-spaces. Show that
the equation
Lu = F'(u).
ue X,
has a solution if the following conditions are satisfied:
(i) The operator L: D(l.) s: X ... X is self-adjoint on the real H-space X,
and the range of L is closed.
(ii) The linearized equation Lu = 0 has a nontrivial solution u  o.
(iii) The functional F: X  IR is convex, continuous. and G-dilTerentiable.
(iv) For all U E X, we have the gro\\.th condition
b
F(u) < 211ull2 + const,
where the constant b satisfies the inequalit). 0 < b < ;" I. Here, A. denotes
the smallest positive eigenvalue of L.
(v)
F( ",.) ... OC;
if II \\' II ... ex:; ,
\\'here w lives in the null space of L.
Hint: The proof is based on a dual variational principle. Cf. Mawhin
( 1986a).

754
29. Noncoercive Equations and Nonlinear Fredholm Altcrnati\'cs
29.14. Positit'e solutions of semilinear elliptic differential equations. We consider the
following boundary value problem:
-14 = f(14) in G.
u > 0 in G.
u = 0 on i1G,
u E C2(G
( 190)
Let
F(u) = f: f(v)dv.
Then the first line of problem (190) corresponds to the variational problem
L 0lDul 2 - f"(u»dx = stationary!.
u = 0 on oG,
( 190.)
where Du = (D. u.. . . .0".14). We assume:
(H) Let G be a bounded region in R'\'. N  2. with smooth boundary, i.e. t
aG E C.
A typical special case of (190) is givcn b)' the following problem:
- 414 = 1'14 4 in G. u = 0 on aGe
u > 0 in G. II E C 2 (G).
where p > 0 is fixed. If q > 1 (resp.O < q < I then problem (191) is called
superlinear (resp. sublinear). We introduce the critical exponent
(191)
_ { CN + 2)j(N - 2)
q"h - + 
for N  3,
for N a I, 2.
29.14a. Typi,'al special case. From our general results below we obtain thc following
special statements. Let N  3 and assume (H). Moreover, let 1J > 0 be given.
Then:
(i) If I < q < qcrit' then problem (191) has a solution.
(ii) If q  q,,11 and G is star-.haped (e.g., G is convex), then problem (191) has
no solution.
(iii) If q  qcril and G has nonrriviallopology (e.g. G is not contractible to a
point if N c: 3), then problem (191) has a solution.
Now, assumc (H) ".jth N = 2. Then qcril = r:J) and problem (191) has a
solution for each q with 1 < q < qCfia'
Finally, let G be an open ban around the origin in R N , N > I. and let q > 1.
Then. each solution u of (191) is radiall" symmetric.
Lack of compactness. The results above show clearly that the critical
exponent qCf'l plays a fundamental role. Note the following crucial fact:
(q Under the assumption (H) with N  3, the embedding
W 2 1 (G) S;; L.+.(G)
;s compact for I S q < qClii and not compact for q = qcrlC'
Roughly speaking, this lack of compactness is responsible for (i) and
(ii) above. In particular, if we consider problem (191), then f(u) = 1141 4 and

Problems
755
hence
1
F(u) = (sgn u)lul.+ I .
q+1
In this case, the functional u.... fa F(u)d. is not weakly sequentially con.
tinuous on the Sobolev space WlCG) if q == qcrlc' This fact is related to (C)
(ef. Step 2 of the existence proof from Problem 29.14g). In Part III we will
study two standard methods for solving variational problems. namely, the
I..justemik-Schnirelman theory and the Morse theory. If q - qnh' then it is
not possible to apply straightforwardl)' t hcse theories to problem (190.), since
the crucial Palais-Smale compactness condition is violated. by (C).
This lack of compactness only appears in R N for N  3. In fact, let G be a
bounded region in AN. If N = 2 Cresp. N = I then the embedding (E) above
is compact for all q with I < q < 00 (resp. I  q < (0),
Critical e.tponents in differential geomelr}'. It is quite remarkable that
important nonlinear differential equations in differential gcometr)' are related
to situation (C) above for q = qClit' For example, this concerns:
(a) the Yamabe problem (cf. Schoen (1984) and the SUf\'ey article by Lee and
Parker (1987»; and
(b) the Yang-Mills equations in gauge field theory (ef. Taubcs (1982).(t982a
(1986, S».
Problems (a) and (b) will be discussed in Chapters 85 and 96, respectively.
Concerning critical exponents for scmilinear partial differential equations,
we also recommend the survey article by Brczis (1986).
29.14b-. Ex;stenL"e theorem for SIIperlinear problems in Ihe noncritical case. Let N > 3.
Along with (H) above, we make the following assumptions:
(H I) Superlinearit y. The function I: R..  R.. is locall y Lipschi tz con tinuous
and superlinear, i,e.. more precisely, we have 1(0) = O.
lim f(u  = 0 and lim f(u) = +00.
If -. . 0 u . - to at) U
(H2) Subfritical gro",th. The function U'-' I(u) does not grow faster than
u uf<Ii' as u  +00. More precisely,
lim f(u) = O.
.. U fut.
U" CI)
and u..... f(u)fut-.. is nonincreasing on ]0, ::tJ[.
(H3) There exists a number q with - I S q < q.:rit such that
uf(u)  (q + l)F(u)
for all u  U o and fixed U o .
Then:
(a) Ex;stence. The original problem (190) has a solution.
(b) A priori estimates. There exists a constant C > 0 such that lIull  C for
each solution u of (190).
Corollary (The simpler two-dimensional case). Assume (H) with N = 2 and
(HI). Then statements (a) and (b) remain true.
Example. Let JJ > O. The assumptions made above are satisfied for the
function
f(u) = IIU f .

756
29. Noncoercive Equations and Nonlinear Fredholm Altemati\'cs
\\'here 1 < q < qrit for J'I  2. Note that F(u) = ,'U 4 " l j(q + I) for 14  0 in
(H 3).
Hint: cr. Dc Figuciredo, Lions. and Nussbaum (1982
29.14c. The noneX;Slellce theorem of Pohoiaet' (1965). Let G be a bounded region in
R''', N  1 with cG E c (i.e., G is a bounded open interval for N = I). Let
f: R. -+ IR be continuous. Show that the following hold.
(i) If U is a solution of the original problem (190), then
2N [ F(u)dx - (N - 2) [ uf(u)dx = r (x - }')n(x) u 2 dO. (192)
JG JG J tn
for each fixed }' E }iN. Hc n(x) dcnotes thc outcr unit normal at the point
x e ijG. Recall that F(u) r:iJ $0 f(v) dv.
(ii) Let N  3. If the region G is star-shaped (e.g.. G is convex) and if
(q'il + I)F(u) :s;; uf(u) for all u  0, (193)
where f(u) > 0 for all II  O. then the original problem (190) has no
solution u.
Exmnple. Let f(u) = /lut for fixed q  q,Ic' Jl > 0, and all u  O. Thcn
f"(u) = IJu"+ I /(q + I) for all u  O. and hcnce condition (193) is satisfied.
Solution: Ad(i). M ultipl)'ing the original equation - Au = f(u) b)'
(. - '7.)D,u, we get
(R) - t (i - "i)DjuAudx = L (j - '1,)D,uf(u)dx.
Here we sum over i,j IiiiI 1,..., N,and we set x = (I"."NY = ("J,...,'1N),
n = (n I' . . . , "N  and D, = (;/o;. \Ve want to sho\\' that (R) implies the desired
relation (192) by simply using integration by parts. In fact. since u =- 0 on cG.
integration by parts yields
r (i - '1i)D , uf(u)dx = r (, - '1,)D,F(u)dx
JG JG
= f (, - tI,)n,F(u(x»dO - r (D,(, - ",»F(u)d.
iG JG
= - r NF(u)dx.
JG
since F(O) = O. Moreover, using II = DJ(DJu integration by parts yields
- L (, - 'Ii)D,uDj(Dju)dx ac: A + B.
whcre
A = - f (. - '7,)D,u(nJD J u)dO,
..'G
B = L DJ[(' - 'l.)D,u]DJudx.

Problems
757
Since u = 0 on cO. the tangential derivatives of u vanish on cG, and hence
Du  I Du III, i.e.,
DJu = IDulnJ on cG
for all j.
( 194)
This implies
A = - LG (, - ",)11 , 1 Dul 2 dO
= - f (x - y)nlDul 2 dO.
i'G
By the product rule..
B = t ["jDjuDjudx + C. where C = L (, - ",)DiDJuDJudx.
Integration by pans yields
C = L (. - "1)II I ID u I 2 dO - t NIDul 2 dx - C.
and hence
c =  t (x - y)nlD u l 2 dO -  L IDul 1 dx.
Finally, integration by parts yields
L IDul 2 dx = L DJuDJudx
= r nJuDJudx - r uAudx = r uf(u)dx.
J('(j JG JG
Putting all these relations together, we get the claimed inequality (192).
Note that IDul 1 = Icu/onl 2 on aG, by (194).
Ad(ii). If the region G is star-shaped, then there exists a point }' e G such
that
(x - }')n(x) > 0
for all x e cG.
(t 95)
Suppose that u is a solution of the original problem (190). Since f(u) > 0 for
all rl  0, we get
4u s 0 in G,
and II = 0 on aG as well as u > 0 in G. Thus, the C 2 -function u: G -+ R attains
its minimum at each point of the boundary oG. By the strong maximum
principle for elliptic equations applied to - u, this implies

ou
 < 0 on oG
on
(cf. Problem 7.2 from Part II). Noting that qCflt + I = 2N/(N - 2), it follows

758
29. Noncoerci\'e Equations and Nonlinear Fredholm Alternatives
from the decisive inequality (192) along with (193) and (195) that au/on = 0
on aGo That is a contradiction.
29.14<1-. The impact of topolog}' on semilinear elliptic equations ,,'ith critical exponent.
We consider the problem
- L\u = pu..... in G,
U 1:1 0 on cG,
u > 0 in G. u e C 2 (G),
where JJ > 0 is fixed. and G is a bounded region in R"', N > 3. with oG E e-s;.
We sa)' that G has nontrivial topology iff there exists an integer k  1 such that
(196)
H1fc-,(G.Q):;: 0 or H 21 - I (G, mod 2)  O.
Here, II.(G, 0) and H",(G. mod 2) denotes the mth homology group of G with
coefficients in (» (the field of rational numbers) and l2 = lj2l. respectively
(cf. Chapter 97). For N = 3. G has nontrivial topology iff G is not contractible
to a point. For N > 4. a large variety of regions G has nontri,'ial topology.
For example, this is the case if G is homeomorphic to a solid torus or G has
a holc.
Show that, for each p > 0.. problem (196) has a solution if G has nontrivial
topology.
Hint: Cf. Dahri and Coron (1988).
29.14e.. Existence theorem in the sub linear case. We consider the problem
- u = pf(u) in G.
u > 0 in G.
u = 0 on (1G,
u E C 2 (G»)
( 197)
Yt'here JI > o. Let S denote the set of all solution pairs (l. u) of (197). Suppose
that f: R  R is C 1 and sublinear, i.e.,
lim f(u) = +00.
..-0 u
Show that the set S u (0.0) contains an unbounded component t in thc
space R x C 1 (G) with (0.0) E I.
Hint: Cf. Turner (1974).
29.14(*, Radially symmetric solutions. Let G be an open ball around the origin in R"'.
l\' > 1. and let f: R  R be e l .
Show that each solution u of(197) is radially symmetric.
Hint: ef. Gidas) Ni. and Nirenberg (1979). The proof is based on the
maximum principle and on an important device of moving parallel planes to
a critical position and then showing that the solution is symmetric about the
limiting plane (ef. also Kawohl (1985, L».
29. 14g*. The importance of best Sobolev constants. Finally. we study the following
problem:
-Au - a(x)u = u. in G.
u > 0 in G.
u = 0 on oG,
u E C 2 (G).
( 198)
In the special case \\-here N  3 and q = qcrit' this problcm is closely related

Problems
759
to the famous Yamabc problem in differential geometry (cf. Chapter 85).
(H I) Let G be a bounded region in N"', N   with aG e CX). Let a E C))(G).
(H2) The smallest eigenvalue PI of the linearized eigenvalue problem
- u - a(x)u = pu in G,
is positi\'c. Recall that
U &:I 0 on cG,
( 199)
· f JG (IDuI 2 - au 2 )dx
P m In --
I MC;W/(Gt-(oJ !lull
( 199*)
In the case where N  3, we also introduce the so.callcd best Sobolev constant
S = inf fG ID12 dx .
... It 1 (G} - (O) Jj u II q... . I
Notc that the embedding Wl(G) S L.".tl(G) is continuous. Hence the
number S > 0 is the smallest constant such that
Ilull;«.., s Sllulli.2.0
Moreover, we define
for all u e J1l(G
J = inf
u II"/IG)- :0)
JG (IDuI 2 - a,,2)dx
1 u lI;",,+-. -
Finall).. for N = 3. let K denote the Green function with respect to the
linearized problem for (198 i.e., for each fixed )' E G, we have
I
K(x, ).) =- 4 _ I I + k(x, }').
nx-}'
where
-d1CK - aK JIII;, in G.
K(x,)') = 0 for all .t E aGo
Hcre.)' denotes Dirac's delta distribution at the point y (ef. A 1 (64)). Observe
that the function k( . . . ) is continuous on G x G.
Remark (Necessary condition). Let q > 1. Then. under the assumption (H I)
above, condition (H2) is necessary for the solvability of the original problem
( 198).
In fact, let u be a solution of (198), and let (U.,I'l) be an eigensolution of
(199). Then u > 0 and "I > 0 on G. Multiplying (198) with U 1 and integrating
by parts. we get
fG u"u.dx - fG (-.6u. - au.)udx.. P. L u.udx.
and hence Jl. > O.
Existence theorem. Show that, under the assumptions (H I) and (H2), the
(ollowing hold:
(i) If N > 2 and I < q < qc,... then the original problem (198) has a
solution.

760
29. Noncoercive Equations and Nonlinear Fredholm Alternati\'es
(ii). If. = 3 and q = q,.., then (198) has a solution provided k(x. x) > 0 for
some x e G.
(iii)$ If N  4 and q = qcrit. then (198) has a solution provided a(x) > 0 for
some x e G.
Corollary. Assume (H I) and (H2). Let N  3. Then:
(a) 0 < J < S.
(b) The best Sobolev constant S is not achieved in any bounded region G.
Moreover, S is independent of G; it depends only on N.
(c) If J is achieved. then the original problem (198) has a solution.
(d) J < S 10 J is achieved.
(e) If l\' = 3, then:
k(x, x) > 0 for some x e G  J < S.
(r) If /\'  4, then:
a(x) > 0 for some x e G  J < S <:> J is achieved.
Hint: a. the survey article by Brezis (1986). The main idea is to solve the
following constrained minimum problem:
minf(u) = J., 14 e 21(G).
g(u) = I.
(200)
where
f(u) = L (lDu1 2 - au 2 )dx.
g(u) = L lul'''' dx.
Obviously.
. r !G(IDuI2 - au 1 )dx
J. = In 2 '
II. WJ1(G)-(O} nu 141
and J 1:1 J f (9tf-
Let us prove statement (i). Let X = W 2 1 (G).
Step 1. Let N > 2 and 1 < q < q'II' Suppose that J. is achieve i.e., the
minimum problem (200) has a solution. We want to show that this implies
the solvability of the original problem (198).
We first show that u 1(14)1(2 is an equivalent norm on X. Since P. > 0,
f(u)  JAlliulii
for all u EX.
For sufficiently small I; > 0 and all u e X.
if(u)  £ r IDul 2 dx - £lIuui ma la(x)l.
JG G
Thus, there is a constant c > 0 such that
feu)  c L (lDu1 2 + u 2 )dx for all u e X.
\Ve now show that J f > o. Otherwise, there would exist a sequence (un) in
X such that
!(U,.)  0 as n  oc and g(U,.) = I for all n.

Problems
761
Hence u" .... 0 in X as n  a:J. Since the embedding X  Lq+, (G) is continuous.
\\'e get g(u,,) ... 0 as n .... OC'. This is a contradiction.
Suppose now that u is a solution of (200). We ma)' assume that u  0 on
G. Otherwise. we pass from u to lul. by using Problem 21.3g. According to
the Lagrange multiplier rule (Proposition 43.6 from Part III), there exists a
number p > 0 such that
f'(u)h = 1l9'(u)h
forall he X,
(20t)
y,'herc
f'(u)h -- 2 L (DuDh - auh)dx,
g'(u)h ::;; (q + I) L uthdx.
In this connection, note that g'(u)u > O. Letting h :::: u, it follows from (201)
that Jl > o. Furthermore, by (201). u is a generalized solution of the following
boundary \'alue problem:
- u - au I: !(q + 1)14' in G. u = 0 on eG. (202)
By regularit)' theory, u is also a classical solution of (202), where u e (.(G),
since a E Coc,(G). Furthermore, since u  0 on G and u = 0 on oG, it follows
from thc strong maximum principlc (Problem 7.2) that u > 0 in G. Otherwise.
we would have u = 0 on G which contradicts g(u) = I.
Consequently, it follows from (202) that the function tu is a solution of the
original problem (198) if we choose an appropriate number t > O.
Step 2. Again let tv > 2 and I < q < qcril' By using a standard compact-
ness argument, we want to show that the minimum problem (200) has a
solution.
Let (14,,) be a minimal sequence in X corresponding to (200 i.e., y,'c
have
1(14,,) ... J. as n -t oc; and 9(14,,) = 1 for all n.
Since u....... f(u) 1/2 is an equivalent norm on X, the sequence (u.) is
boundcd in X. Thu thcre cxists a subsequcncc. again denoted b)' (u.
such that
Un .... u in X as u -t XJ
(203)
and
I(u) S lim feu,,).
,,-
Since the embedding X s; Lq+1 (G) is compact it follows from (203) that
g(u,,) -+ g(u)
as n 4 C().
Hence f(u) &I J" and g(u) ;II; I, i.e., u is a solution of (200).
From Steps I and 2 we immediately obtain assertion (i).
In thc critical case N > 3 and q = q'i" Step 1 above remains valid. since
the embedding X  L,,+a(G) is continuous for q :; qni'. Consequently. in
order to prove assertions (ii) and (iii) above, it is sufficient to show that J is
achicvcd. In this connection. we refer to BrCzis (1986. S).

762
29. Noncocrcivc Equations and Nonlinear Fredholm Altcrnati\'cs
References to the Literature
Classical "'orks: Fredholm (1900) (integral equations). Smale (1965) (nonlinear
Fredholm operators). Pohoiae\' (1967) (nonresonancc case). Landesman and La1r
(1969), Ambrosetti and Prodi (1972) (global multiplicity theorem). Ambrosctti and
Rabinowitz (1973) (variational methods).
Introduction: Nirenberg (1974, L), (1981, S). Berger (1977 M), Fucik and Kufner
(1980, M), Fuik (1981, M).
Nonlinear Fredholm alternatives: Pohoiaev (1967 Landesman and Lazcr (1969).
Kaurovskii (1970), Nirenberg (1970), (1974. L) Hess (1972). (1974a), Cronin (1973
Schechter (1973), (1978), Fuik, Netas. and SouCek (1973, L). Neeas (1973). (1983. L
Berger and Podolak (1974), (1975), Kazdan and Warner (1975), Pctryshyn (1975, S
(1980 (1981), (1986, S), Browder (1978), (1986, p Pascali and Sburlan (1978. M).
Nonlinear Fredholm alternatives for general semilinear elliptic equations: Nirenberg
(1974, L) (recommended as an introduction). Nirenberg (1970). Schechter (1973), Berger
and Schechter (1977).
Stable homotopy and nonlinear Fredholm alternatives: Nirenberg (1974, L) (recom-
mended as an introduction Nirenberg (1970), Cronin (1973), Berger and Podolak
(1974 (1975), Berger (1977, M).
Stable homotopy and the topological classification of nonlinear Fredholm maps:
Svarc (1964), Berger (1977, M Booss and Bleecker (1985, M) (K-theory and the
Atiyah-Janich theorem).
Nonlinear Fredholm maps: Berger (1977, M), Borisovif (1977, S).
Bifurcation theory for potential operators: BOhme (1972), Rabinowitz (1974. S).
(1977), Chow and Hale (1982, M) (see also the References to the Literature for Chapter
45).
Stability in nonlinear elasticity: Beckert (1976), (1984, S).
Semicocrcive problems: Hess (t 974a).
Variational methods for semilinear elliptic equations: Rabinowitz (1986. L).
Positivity methods for semilinear elliptic equations: Pohobe\' (1965) (nonexistence
theorem Turner (1974 Amann (1976, S) and Lions (1982, S) (important survey
articlcs De Figueiredo, Lions, and Nussbaum (1982).
Critical Sobolev exponents and positive solutions of semilinear elliptic equations:
Brezis (1986, S) (important survey article Schoen (1984), Bahri and Coron (1988) (the
impact of topology).
Radially symmetric solutions of semilinear elliptic equations: Gidas, Ni, and
Nirenberg (1979).
Symmetrization and rearrangements: Kawohl (1985, L).
Existence theorems for quasi-linear elliptic and parabolic equations via subsolutions
and supcrsolutions: Deuel and Hess (1975), Deuel (1978).
Combination of different methods: Hofer (1982
M ulliplicit), results for scmilinear elliptic equations: Ambrosctti (1983, S) and Ruf
(1986, S) (recommended as an introduction Ambrosetti and Prodi (1972 Ambrosetti
and Rabinowitz (1973), Nirenberg (1974, L Rabinowitz (1974, S), (1986, L), Kazdan
and Warner (1975), Amann (1976, S Hess (1981 Hofer (1982), Lions (1982, S De
Figueiredo and Solimini (1984), Berger (1985). Benkert (1985). (1989), Rabier (1986
Range of sum operators and applications to scmilinear equations: BrCzis and
Haraux (1976), Brezis and Nirenberg (1978).
Alternative problems. coincidence degree, and ordinary differential equations:
Cesari (1964), (1976, S), Mawhin (1972 (1979, L Gaines and Mawhin (1977, L, S, H).
(Cf. also the References to the Literature for Chapters 7 and 49 concerning scmilinear
differential equations.)

References to the Literature
763
Nonlinear differential equations in differential geometry: Yau (1986, S) (fundamental
survey article), Yau (1978) (complex Monge-AmpCre equations and the Calabi prob-
lem), Schoen and Yau (1979) (positive energy theorem in general relativity), Sacks and
Uhlenbeck (1981) (minimal immersions of 2-sphcres). Hamilton (1982a Taubcs (1982),
(1982a), (1986), Uhlenbeck (1982), as well as Freed and Uhlenbeck (1984, L) (Yang-
Mills equations in gauge field theory); Schoen (1984) (1986. S) and Lee (1987, S)
(solution of the Yamabc problem-conformal deformation of a Riemannian metric
to a constant scalar curvature), Kazdan (1985, L) (prescribing the curvature of a
Riemannian manifold), Hildebrandt (19848, S). (1986. S) (minimal surfaces). (1985, S)
(harmonic mappings between Riemannian manifolds Wente (1986) (counterexample
to the Hopf conjecture), Jost (1984, L), (1985. L), (1988. L), (1988a. S) (harmonic
mappings and minimal surfaces).
Physics and geometry: Witten (1986, S) (string theory Piran and Weinberg (1988,
L) (superstrings), Atiyah and Hitchin (1988, L) (magnetic monopoles).
(Cr. also the References to the Literature for Chapter 85 (Riemannian geometry) and
Chapter 96 (gauge field theory).)

GENERALIZATION TO NONLINEAR
NONSTATIONARY PROBLEMS
The strides that have been made recentl)', in the theory of nonlinear partial
differential equation are as great as in the linear thcol)'. Unlike the linear case,
no wholesale liquidation of broad classes of problems has taken place; rather,
it is steady progress on old fronts and on some new oncs, the complete solution
of some special problems, and the discoycry of some brand new phenomena.
The old tools-variational mcthods, fixed-point theorems, mapping degree,
and other topological tools have been augmented by some new oncs. Pre-
emincnt for disco\"cring new phcnomcna is numerical cxperimcntation; but it
is likely that in the future numerical calculations will be pans of proofs.
Peter Lax (1983)
In Chapters 30 through 33 we want to apply the following methods to
nonlinear evolution equations:
(i) the Galerkin method;
(ii) the method of nonlinear semigroups: and
(iii) the method of maximal monotone operators.
Chapter 32 is devoted to a detailed study of the properties of maximal
monotone operators, which play a key role in the theory of monotone
operators.
The study of abstract nonlinear evolution equations will be continued in
Part V in connection with applications to important problems in mathe-
matical physics.
765

CHAPTER 30
First-Order Evolution Equations and
the Galerkin Method
Use se\'eral function spaces for the same problem.
The modern strategy for nonJinear
partial differential equations
In this chapter, we generalize the Hilbert space methods in Chapter 23, Cor
the investigation oC linear parabolic differential equations, to nonlinear prob-
lems. In this connection, as an essential auxiliary tool, we use the Galerkin
method.
30.1. Equivalent Formulations of First-Order
Evolution Equations
The goal of this section is to present various equivalent formulations of
first-order evolution equations. This is useCul with a view to both abstract
existence proofs and applications to nonlinear parabolic differential equa-
tions. Let "V s; H s; V." be an evolution triple.
30.1a. The Original Problem
We consider the following basic initial vallie problem:
1l'(I) + A(t)ll{t) = b(t)
for almost aU t E ]0, T[,
(1 a)
(I b)
(Ie)
11(0) = "0 e H,
II e W,I (0, T; V, H), I < p < 00.
767

768
30. First-Order Evolution Equations and the Galerkln Method
For each, E ]0, T[, let b(r) E V* and let A(t) be an operator of the form
A(t): V -+ V..
For given "0 e H, we seek a function u: [0) T] -+ V such that (1) is satisfied.
Recall) from Section 23.6, that condition (Ie) means that
u E Lp(O, T; V») u' E Lq(O, T; V*), p-l + q-l = I,
where the derivative u' is to be understood in the generalized sense.
The initial condition (I b) is meaningful because it follows from Proposition
23.23 that the embedding W p 1 (0, T; V, H) c C([O, T], H) is continuous. More
precisely, after a modification of the function u E W p . (0, T;  H) on a subset
of [0, T] of measure zero, if necessary, we obtain a uniquely determined
continuous function u: [0, 7.] -+ H. Condition (I b) is to be understood in this
sense.
30.1 b. The Functional Equation
Equation (la) is equivalent to
(u'(t), v)., + (A(t)u(t), v)., = (b(t), v)v,
(2)
for all v e V and almost all t e ]0, T[. To be precise, we assume that there
exists 8 subset Z of ]0, T[ of measure zero such that (2) is valid for all v e V
and for all t e ]0, T[ - Z. Note that Z is independent of v.
For all u, v e Jt: t e ]0, T[, we set
aCt; u, v) = (A(t)u, v)."
bet; v) = (b(t), v)v.
(3)
By Proposition 23.20, the original problem (1) is eq"i(,/ent to the following
p' oblem. We seek a function u such tha for all v E V and almost allt E ]0, T[,
d
dt (u(t)lv)s + aCt; u, v) = bet. v).
(48)
u(O) = Uo E H, (4b)
u e W p 1 (0, T; J': H), I < p < 00. (4c)
In (4a), dldt is to be understood as the generalized derivative, i.e., to be precise,
(4a) means
- foT (u(t)1 v)"tp'(t)dt + t T aCt; u(t). v)tp(t) dt
= foT bet; v)tp(t)dt for all tp e CoCO, T), v e V.
One can easily carryover concrete nonlinear parabolic equations by means
of integration by parts into problems of the form (4). We discuss this in Section

30.1. Equi\'alcnt Formula(ions of First-Order Evolution Equations
769
30.4. In this connection, there will result immediately a(t; u, v) and bet, v) from
the differential equation under consideration. By means of (3), one has then
to construct A(t) and bet). The explicit time-dependence of a( · ) and hence of
A(') is due to the time-dependent coefficients, and b(.) results from the right
member of the differential equation.
30. Ie. The Galerkin Equations
Let {"'I' ,v 2 ,...} be a basis in  We set
.
Un(l) = L Ct,,(t)w t .
t-I
Motivated by equation (2), we define the Galerkin equations in the following
way. For almost allt e ]0, T[, we seek a function u" such that
<u(t), "i)v + (A(t)u,,(t), "'i)., = (b(t), WJ)V, j = 1,..., n, (Sa)
u,,(O) = "..0 e H., (Sb)
U" E L,(O. T; H,,), u e L.,(O, T; H,, (5c)
where Hit = span {"'I"'" ,vn}' and the derivative u is to be understood in the
generalized sense.
Using the relations (3) and (4), the Galerkin equations (5) can be written
in the following explicit form:
r. cn(r)("il W})H + a ( t; f c'u,(t)"i. Wj ) = bet, Wj), (6a)
.=1 t-l
Cj,,(O) = rljO' j = It..., n, (6b)
for almost all t e ]0, T[, where the initial values rljO are given.
Since the 'VI' . . ., '\'" are linearly independent, we get
det«\\il'''J)H)  0,  j = 1,..., n.
Therefore, the system (6a) can be solved for the derivatives c. Henc the
Galerkin equations (6) represent a first-order system of ordinar)J differential
equations for the real functions t...... cu(t) on ]0, T[, where k = 1, .. ., n.
In Section 30.4 we shall encounter the Galerkin method in the form (6), in
connection with nonlinear parabolic equations. However, the coordinate-free
form (5) is more convenient for the proof of convergence for the Galerkin
method.
30.1d. The Final Operator Equation
We set
x = Lp(O, T; V),

770
30. First-Order Evolution Equations and the Galerkin Method
and hence X* = Lq(O, T; V*), by Section 23.3. Moreover, we set
(AI4)(t) = A(t)u(t) for all t e ]0, T[.
In Theorem 30.A below, we shall show that this defines an operator of the form
A: X -+ X*,
and the original problem (1) is equivalellt to the following operator equation:
14' + All = b,
(7a)
(7b)
(7c)
u(O) = 1'0'
ueX, u'eX.,
where 1'0 e Hand b E X. are given. Recall from Section 23.6 that (7c) is
equivalent to II E W p 1 (0. T: V, H).
Problem (7) is undoubtedly the most elegant form of a first-order evolution
equation. However, for concrete parabolic differential equations, one arrives
at (7) only via (1)-(4). For that reason, we have devoted so much attention to
thc various formulations of the original problem (1).
30.2. The Main Theorem on Monotone First-Order
Evolution Equations
We summarize our assumptions:
(H 1) Er.,-olution triple. Let .. V c H  V." be an evolution triple with dim V =
00. and let {"'., 'V2'. . .} be a basis in V. We set
H" = span {"'I'. · · , w n }
and introduce on the II-dimensional space H. the scalar product of the
H-space H. Notc that H" s V s H.
Let the sequence (u"o) be an approximation of the given initial value
140 e H, i.e., suppose that
U ItO -. 14 0 in H
as n -+ 00.
Let 0 < T < 00, 1 < p < 00, and p-I + q-I = 1.
(H2) Monotonicity. For each t e ]0, T[, the operator
A (t): V -. V.
is monotone and hemicontinuous.
(H3) Coerciveness. For each t E ]0, T[. the operator A(t) is coercive, i.c., more
precisely, there exist constants c( > 0 and C2  0 such that
(A(t)t\ v)v > c1llvllt - C2
for all v e V, I E ]0, T[.

30.3. Proof or the: Main Theorem
771
(H4) Gro\vtlJ condition. There exist a nonnegative function "3 E L,(O, T) and
a constant C4 > 0 such that
IIA{t)vllv.  C3(t) + c4I1vll-1 for all ve J': t e ]0, T(.
(H5) Measurabilit}r. The function t...... A(t) is weakly measurable, i.e., the
function
t ...... (A (t) u, v) v
is measurable on ]0. T[. for all u. v e 
(H6) Let Uo E Hand b E L.(O, T; V*) be given.
In Section 30.4 we shall show that the assumptions in the present form can
easily be verified for nonlinear parabolic equations.
Theorem JO.A. Assume (HI)-(H6). Then:
(a) Existence and uniqueness. The original initial value problem (1) has a
unique solution u.
(b) Convergence of the Galerkin method. For each n = 1, 2, ..., the Galerkin
equatiolJ (5) has a unique solution u ll . The sequence (u ll ) converges to the
solution u of (I) in the followillg sense:
UIIU in Lp(O, T; V)
max lIu.(t) - u(t)II" .... 0
OSIsT
as n -+ oc,
(8)
(9)
as n .... 00.
(c) Equivalent operator equation. Lel X = L,(O, T; V). If "'e set
( Au)(t) = A(t)u(t) for all t e ]0, T[,
the" the operator A: X --. X. is mOlJotone, hemicontinuous, coercive, and
boulJded. TIle original initial value problem (I) is eqllivalent to the operator
equation (7).
30.3. Proof of the Main Theorem
If a man.s wit be wandering, let him study mathematics. for in demonstration,
if his wit be called away ever so little he must begin again.
Lord Bacon (1561-1626)
We first summarize several auxiliary tools. In order to simplify the writing
of formulas, we agree to use the follo\\'ing abbreviations:
(u, v) = (u, v)v,
(Ill v) = (ulv)H'
nu = lIull y ,
lul = lIullH'

772
30. First-Order E\'olution Equations and the Galerkin Method
By (23.25), the integration by parts formula
(u(t)lv(t)) - (u(O)lv(O» = f (u'(s), v(s» + (v'(s), u(s» ds (10)
holds for all u, (,' E W,J (0, T; It: H) and for all t E [0, T]. This formula forms the
decisive ba.i. for our proof together with the monotonic;t}: trick in Lemma
30.6 below.
Furthermore, for all II e X., veX, and 0 s: t S T, we have that
UulI1-. = f: lIu(t)II. dt, Ilvll = f: II v(t)II" dr,
(u, v)x = IT (u(t v(t» dt
(11)
and
f (u(s), v(s»ds s; f I(u(s v(s»lds
s lIullx.Jlvllx,
(12)
where (12) follows from the Holder inequality. In the sense of the identification
of Convention 23.14, we obtain that, for all u e H, veil:
(u. v) = (ulv).
IIvllv.  constll'J  constllvll.
(13)
Lemma JO.I. Suppose that either
U lt -. u in X. and v v in X as n -. 00,
.
or
II u ;11 X. and v. -+ V in X as n -+ 00.
"
T/ren. for all t e [0, T], as II -+ 00:
f (Un(S), VII(S» ds..... f (U(S), v(s» ds.
(14)
This follows from Proposition 23.9. Furthermore, for each n e N,
Hit S V 5 H  V.,
and
IIvll, II vII v. s: clvl for all v e Hit and fixed c > 0, (1 S)
since dim Hit < 00, and all norms are equivalent on tinite-dimensional B-
spaces. Therefore, it follows from
Un E L,(O, T; H.),
U E L 4 (0, T; Hit)'

30.3. Proof or the Main Theorem
773
that
u. e Lp(O, T; V),
and hence u" E W,l (0, T; V. H).
U E L 4 (0. T; V.),
(16)
30.3a. Proof of Uniqueness
We first prove the uniqueness of the solution U of the original problem (1). Let
U, v e W p l (0, T; V, H) be two solutions of (I). Using integration by parts and
the monotonicity of the operator A(s) for all S E ]0, T[, we obtain that, for all
t e [0, T],
lu(t)-v(t)1 2 -lu(O)-v(O)I Z =2 f (u'(s)-v'(s). u(s)-v(s»ds
= - 2 f (A (s)u(s) - A (s)v(s). u(s) - v(s) > ds  O.
Thus, from u(O) = v(O) we get "(1) = v(r) for aliI e [0, T].
We now prove the uniqueness of the solution u" of the Galerkin equation
(5). To this end, let u. and v" be two solutions of(5). Then, U". v" E Wi (0. T; J1: H),
and
(u(t) - v(t). U,,(I) - vn(t» = - (A(t)I'n(t) - A (t)vlI(t), "n(t) - vlI(t).
As above, this implies U,,(I) = vn(t) for all t e [0. T].
o
30.3b. The Equivalent Operator Equation
We \\'ant to prove Theorem 30.A(c). Recall that
X = Lp(O, T; V). X. = Lq(O, T; V*).
For each u e X. we set
(Au)(I) = A(t)u(t)
for all t e ]0. T[.
Lemma 30.2. For all u EX. v E  the real function
t..-. (A(t)u(t), v)
;S measurable on ]0, T[.
The proof will be given in Problem 30.1. Since ,. V c: H s;; V." is an evolu-
tion triple, the B-space V is reflexive. Therefore, Lemma 30.2 and the Pettis
theorem A 2 (JO) imply that. for each U e X. the function
t...... A (t)u(t)
is measurable from ]0, T[ to V*.

714
30. First-Order E\'olution Equations and the Galerkin Method
(I) BoundedlJe.s. We show that the operator A: X -+ X. is bounded. Let
u e X. Noting plq = p - 1 and the inequality A2(30b) it follows from
the growth condition (H4) that
IIA(I)U(I)II. S const(lc3(t)l. + Ilr4(t)IIP) for all t E ]0, T[, (17)
By A 2 (9), the real function t .....IIA(t)u(l.)II. is measurable on ]0, T[.
Since C3 E Lq(O, T) and u e L,(O, T; V), the function on the right-hand
side of(l7) is integrable over ]0, T[. By integration, we obtain from (17)
that
IIAulix.  const( IIc) 114 + lIull4) for all U EX,
recalling that II Au II. = J III A(t)u(t) 1I. dt.
(II) J\fOlJOtOlJ;c;t)'. We show that A: X  X* is monotone. Let u, VEX. Since
Au E X*, the Holder inequality (Proposition 23,6) tells us that the real
function
t t-+ (A(t)r4(t), v(t»
is integrable over ]0. T[, From the monotonicity of A (t): V  V. for each
t E ]0, T[, it now follo\vs immediately that
(All - Av. u - v)x = f: (A(I)II(t) - A(t)v(I). U(I) - v(t» dt > 0,
for all u, v EX.
(III) Coercit'elJes.. The operator A: X -. X. is coercive, since
(Au. u)x = foT (A (I)U(t). u(t» dt
> c 111 u II  - "z T for all u EX, ( 18)
by assumption (1-13). This implies that
(Au, u)x/llullx  00 as lIuli x -+ 00.
(IV) Hem;continuil)'. Finally, we show that A: X -. X. is hemicontinuous, Let
u, v, W E X and 0  l, Jl  1. For all t E ]0. T[, it follows from (17) and
the inequality A 2 (30b) that
1< A(t)(u(t) + Av(r», w(t» I
 const(lc3(1)1 + IIU(I) + lv(t)II"q) II \\1(t) II < met),
\vhere
met) = const(lc3(t)1 + lIu(t)II"q + II v(I)II'/4) n\v(t)lI.
Because of c 3 E L.(O, T), and hence C J ELI (0, T), and because of u, V,
M' E Lp(O, T; V) it follows that
II u( · )11 p,rq, II v(' )1) pl. e L,(O, T) and II w( ')IJ E Lp(O, T).
By the Holder inequality, the majorant function m(.) belongs to L 1 (0, T).

JO.3. Proor of th Main Theorem
775
Therefore, it follows from the principle of "Jajorized cont'ergence A 2 (19)
that
lim (A(u + lv w)x = lim i T (A(t)(II(t) + ).v(t)), w(t)xdt
A,-',. A-III 0
= (A(u + pv), "'>x,
which shows the hemicontinuity of A.
(V) Eqllit\Qlence. The equivalence between the original initial value problem
(1) and the operator equation (7) follows immediately from our con-
siderations in Section 30.1.
The proof of Theorem 30.A(c) is complete.
o
30.3c. Proof of Existence
Step I: A priori estimates for the solutions U lt of the Galerkin equations (5).
Lemma JO.3. There exists a constant C > O. independelJI of n, such thilt, for all
II e N and all solutions u.. of (5), tile follo\v;ng are valid:
lIu..1I x S C,
ItAu..li x .  C,
max IU,,(I)I s c.
os,s T
(19)
(20)
(21)
PROOF. Ad(19). Since A(t) is monotone, we obtain that
(A(I)Un(l) - A(I)(O), rl,,(t) > 0 for all t e ]0, T[.
Integration by parts yields
!(lII.(t)1 2 - 111,,(0)1 2 ) = f (II(S). 11,,(5) ds
= f (b(s) - A (S)II,,(S), IIII(S) cis (22)
 f (b(s) - A (s) (0), II.(S) ) ds for all t e ]0, T[,
noting (10) and u" e W,I (0, T; V, H). Because u..(O) -. U o in H as n -. 00, there
is a number a such that
! lu.(0)1 2 < a
for all n EN.
Therefore, by (22),
- a  !(lu..(T)1 2 - 111,,(0)1 2 ) = <b - Au", u,,) x.

776
30. First-Order Evolution Equations and the Galerkin Method
By the coerciveness condition (18), this implies
- a  IIbiLt. Jlu"lIx - C 1 Du"l1 + Tc 2 ,
and hence we obtain the assenion ()9 since p > I and the real function
g() = 11b"x. - cllIP + TCl
goes to -00 as  -. +00.
Ad(20). This follows from (19) and the boundedness of the operator
A: X -. X*.
Ad(21). Noting (22) and the Holder inequality (12 we get
!(lu.(t)1 2 - lu,,(0)1 2 ) s lib - A (0) IIx.l1u"lIx.
This implies (21), since 111,,(0)1 2 < 2a for all n. 0
Lemma 30.4. For each n e N, the Galerkin equation (5) has a uniqlle sollltiolJ U II .
This follows from the existence theorem of Caratheodory for ordinary
differential equations in R- (cr. A 2 (61 »). The details will be given in Problem
30.3.
Step 2: Weak con\'ergcnce of a s,4bsequence of the Galcrkin sequence (II,,) in
the space X to a solution II of the original problem (1).
The spaces X, X., and H are reflexive. Therefore, by the a priori estimates
(19)-(21), there exists a subsequence, again denoted by (u,,), such that
U II  u in X and Au.  ". in X. as n -. XJ, (23)
u"(T)z in H as" -. 00. (24)
Recall tha by assumption (H 1),
11,,(0) -+ "0 in H
as n -+ oc.
Lemma 30.5. The limit elements u, ,v, and z sati.Y}t:
u' + '''' = b, u e W,l (0, T; V. H),
u(O) = uo, u(T) = z.
(25)
(26)
PROOF. The point of departure is the formula
(zl"'(T)v) - (uoll/l(O)v) = fOT (b(l) - W(I)J I/I(I)V) + (""(I)V J U(I» dl. (27)
for all t/1 E CXJ[O, T], veil: which we shall prove below, by using integration
by parts and a limiting process.
Ad(2S). As a special case of (27), we obtain that
f: (b(l) - wet), U)"'(I) dt = - foT (u(I)lv)""(I) dt, (28)

30.3. Proof of the Main Theorem
777
for all.p E Co(O, T), v e  Note that <V,Il(t» = (vlu(t» by (13). According to
Proposition 23.20, equation (28) tclls us that the generalized derivative u' exists
and u' = b - \v. Since b, '" E X., we get u' E X.. Moreover from II e X and
u' e X* it follows that u E W p ' (0, T; It: H).
Ad(26). The integration by parts formula (10) yields
(Il( T)I t/1( T)v) - (11(0)1 t/1(O)v) = foT (U'(I), t/1(t)v) + (cjI'(t)v, 1'(1)> dt,
for all .p E C[O. T], veil: Since u' = b - "' it follo\vs from (27) that
(u(T)lt/I(T)v) - (u(O)I.p(O)v) = (zl.;(T)v) - (uolt/l(O)v)
for all t/I e c [0, T], L' e 
In particular, if we choose a function t/1 with t/I(T) = 1 and 1/1(0) = 0, then
(u(T) - zlv) = 0 for all v e 
Since V is dense in H, this implies u(T) = z.
Analogously, if we choose t/I with t/I(T) = 0 and t/I(O) = I, then we get
u(O) = 110'
Ad(27). First let '" e CX\[O, T] and v E H",. Then v e W p 1 (0, T; V, H). For
n > II', the integration by parts formula (10) yields
(u,,(T)I.;(T)t') - (u,,(O)It/1(O)v)
= foT (u(t), cjI(t)v) + (cjI'(t)v, ",,(I» dt.
By the Galerkin equation (5),
(u..(T)Jt/1(T)v) - (u,,(O)I.p(O)v)
= foT (b(I)- A(I)u,,(I),cjI(l)v) + (cjI'(t)v,u,,(f»dt
= (b - Au",t/lv)x + <t/1'v,u,,)x'
Letting n ..... r:fJ and noting (23) (24), we obtain that
(zl t/I( T)v) - (rlol t/1(O)v) = <b - w, t/lv>x + <t/1' v, u) x
for all v e U H",.
"'
Since U. H", is dense in V by (H 1), we get (27) for all v e It: In this
connection, note the following. For each v E V. there exists a sequence (vJc) in
U. H", such that
Vi -. v in V as n -+ 00.
This implies "'va -+ .pv in X = Lp(O, T; V) and .p'Vi ... .p' v in X. = Lq(O. T: V*)
as k ... OCt since the embedding V c V* is continuous. 0
Lemma 30.6. 1'he limit elements u and '" jron, (23) satisfy the equation Au = \\'.

778
30. First-Ordcr E\'olution Equalions and the Galerkin Method
PROOF. We will use a l}'pical argument of the theory of monotone operators.
By (23).
UnU in X as n -. 00,
AUnw in X. as n... 00.
Below we show that
lim (Au n , u,,)x  (,,,.,ll>X.
(29)
,,ao
The operator A: X -. X. is monotone and hemicontinuous. Therefore, it
follows from the fundamental nlonotonic;ty trick (25.4) that
Au = "'.
We now prove (29). The integration by parts formula (10) yields
!(lu..(T)1 2 -lu n (0)1 2 ) = f: (u(t).un(t»dt
= foT (b(t) - A(t)u,,(t) u,,(t) > Jr.
noting the Galerkin equation (5). Hence
(Au..u,,)x = (b,un>x + !(lu n (O)1 2 -1u,,(T)1 2 .
By Lemma 30.5,
(30)
U,.(O) -. u(O)
and hence
and
u,,(T) u(T) in H
as n -. cx::,
IIl(T)1 s lim lu,,(T)I.
"  C()
Since u,,U in X as n -. 00, (b,u,,)x -. (b,u)x. From (30) we get
lim (Aun,u.)x S (b,u)x + !(lu(O)1 2 - lu(T)1 2 ). (31)
"  «,
Finally, integration by parts yields
1(1u(0)1 2 -lu(T)1 2 ) = - foT (u'(t),u(t»dt
= -(u'tu>x = <'" - b,u)x. (32)
by Lemma 30.5. From (31) and (32) \\'e get (29). 0
From Lemmas 30.5 and 30.6 we obtain that the limit element u in (23)
satisfies the following operator equation:
II' + All = b, U e W p 1 (0, T; Jt: H),
u(O) = Uo.
This equation is equivalent to the original equation (1).
Therefore, the proof of Theorem 30.A(a) is complete.
o

30.4. Application to Quasi-Linear Parabolic Differential Equations or Order 2m 779
Step 3: Weak convergence of the total Galerkin sequence (un) in the space
X ::: Lp(O, T; V) to the solution U of (1).
According to Step 2, it always follows from the convergence
14..,  v in X
as n -. 00,
of an arbitrary subsequence of (u,,) that v is a solution of the original
problem (1). Since this solution is uniquely determined, we get v = u. By
Proposition 21.23(i), the total sequence (u,,) converges, i.e.,
u..u in X
as n  00.
Step 4: Convergence of (u lI ) in the space C([O, T]; H).
Lemma 30.7. As n -. 00, maxos.s T lu..(t) - u(t)l-. O.
The proof of this lemma will be given in Problem 30.4. This finishes the
proof of Theorem 30.A. 0
30.4. Application to Quasi-Linear Parabolic
Differential Equations of Order 2m
Let QT = G x ]0, T[. We consider the following initial-boundary value
problem:
u,(x, I) + L(t)u(x, t) = f(x, t) on QT,
D'u(x, t) = 0 on oG x [0, T] for all fJ: IfJl  m - 1, (33)
u(x,O) = uo(x) on G,
where
L(I)U(X, t) = L (- 1 y«1 D 1 Acr(x, DI'(x, t); I)
I Sift
and Dr' = (D'u11 s... Note that the derivatives lJI and D' refer to the spatial
variable x. Thus, for each fixed time t, the differential operator L(I) is of order
2"1 with respect to x.
Our goal is to prove the existence of a generalized solution for (33). In
Section 26.5, we proved the existence of generalized solutions for quasi-linear
elliptic equations. Roughly speaking, we will suppose in this section that, for
each fixed time I, the operator L(t) is a quasi-linear elliptic operator of the
form considered in Section 26.5. To be precise, we assume:
(AI) Let G be a bounded region in IR'''', N  1. We set
V = Wp'"(G), H = Lz(G), 2 S P < 00.
Moreover, let 0 < T < 00, m = 1,2, ..., p-l + q-l = 1.
(A2) For each fixed t e ]0, T[. the differential operator L(t) satisfies the
assumptions (H 1) through (H4) of Proposition 26.12, where we assume

780
30. First-Order Evolution Equations and the GaJcrkin Method
that all the constants and the real functions 9 and h that appear in (H 1)
through (H4) are independent of t.
Recall that (H I) through (H4) concern the Caratheodory condition,
the growth condition. the monotonicity condition, and the coerciveness
condition for the functions Am.
(A3) The real function
t ....... a( I; v, \v),
introduced in Definition 30.9 below, is measurable on ]0. T[, for all v,
\". e JI:
This weak assumption means that the functions Aar depend on time l
in a reasonable manner.
Because of the growth condition (H2) assumed in (A2), the assumption (A3)
is satisfied if all the functions All arc measurable, that is. for each «, the real
function
(x, D, t).-. A\1(x, D, t)
is measurable on G x !lid X ]0, T[.
(A4) Let Ie Lq(Qr) and "0 e L 2 (G) be given.
(AS) Let {"'1' \V2'...} be a basis in V = W,"'(G). We set
H,. = span {"'1'...' \v n }.
Moreover, let (unO) be a sequence in H = Lz(G) such that ".0 -+ Uo in H as
.
n -+ 00, I.e.,
fG (uno(x) - UO(.'t»2 dx -+ 0
as n -. 00.
EXAMPLE 30.8. In the case where m = I, the assumptions (AI)-(A3) are satis-
fied for the following generalized heat equation:
,V
", - L D,(IDtul'-Z Dill) = f on QT'
'el
" = 0 on 00 x [0, T], (34)
u(x,O) = uo(x) on G.
where .'( = (I'...' .v) and D. = %.. By Definition 30.9 below,
a(t; \v, v) = .r t I Djw(x)IP- 2 D,w(x)D,v(x)dx,
G i-I
for all v, \\. e II: Here, a( · ) is independent of t.
PROOf. This follows from the proof of Proposition 26.10. 0
Definition 30.9. The generalized problem associated with (33) reads as follows.
Let Ie Lq(QT) and "0 E Lz(G) be given. We seek a function II e W p 1 (0, T; V, H)

30.4. Application to Quasi- Linear Parabolic Differential Equations of Order 2m
781
such that, for all.) E V and almost all t E ]0, T[,
d
dt (lI(t)1 (.')" + aCt; Il(t), v) = bet; v),
u(o) = "0.
(35)
where
a(l: "".l,) = J. > A«(r. D\\'(x); I)v(:()d,.
(;  III
bet; v) = fG f(x, t)v(x) dx for all v, w e V, t e ]0, T[.
Recall that
(lI(t)\t')H = fG u(x. r)t.(x) dx.
In (3S dldl denotes the generalized derivative on ]0, T[. Therefore, equa-
tion (35) means explicitly
- foT (Il(t)lt.)" tp'(r) dr + foT a(l; U(I). V)tp(l) dl
= foT b(t;v)tp(t)dt for all tp e COO(O, T).
Formally, one obtains the generalized problem (35) by multiplying the
original problem (33) by the function v E Ccf(G) and then integrating by parts
with respect to the spatial variablc x.
The Galerkitl "Jethod for the original problem (33) follows immediately from
the generalized problem (35). We seek a function
n
uIICt) = L CA,,(t)\VA;
1:=1
such that, for almost all t E ]0, T[,
d
dl (u..(r)1 "),, + aCt; u,,(r). "J) = b(r; w).
u.(O) = 'lItO,
j = 1,..., n.
(36)
lIft E L,(O, T; II..),
" e Lq(O, T; H,,).
If \\'C set
"
Ullo = L cr lft "'i.
A:1
then (36) represents a first-order system of ordinary dYJerential equations for
the unknown real functions t  Ctll(l) with the initial condition Cb(O) ::: kn for
k = I,..., n.

782
JO. First-Order E,'olution Equations and the Galerkin Method
Proposition 30.10 (Solution of the Initial-Boundary Value Problem (33)).
AssunJe (A I)-CAS). Tllen Ille generalized problem (35) corresponding to the
origi'Jal problenl (33) is equivalent to equation (I), and all the assul1lptions of
Theorem 30.A are fulfilled. Therefore, all the assertions of Theorenl 30.A are
also valid. In particular, the follo,,,ing hold:
(a) Problem (35) has a unique solution II.
(b) For each n e N, the Galerkin equation (36) has a unique solution U II ' and (u ll )
co"verges to u in the .ense of
max f. (u.(x, t) - u(x, t)2 dx -+ 0
OSIST G
as n -+ 00.
Corollary 30.11 (Properties of the Solution u). B)' Proposition 23.23, tile
so/rlt;on rl e WpJ(O, T; II: H) belongs to the space C([O, T];H), that is,
lim f. (u(x, I) - u(x, S»2 dx = 0 for each s E [0, .f].
r.s G
l1Jerefore. the function t H; u(x, t) is cOIJtinuous on [0. T] in tIle mean.
B}' the constructiOIl of tI,e space W,I (0, T; II: H), the functioll x H u(x, t)
belolJgs to the Sobolev space V = Wp.(G), for almost all t e ]0, T[, i.e., this
junctiolJ lias gelJeralized deril,atives up to order m ,,'ith respeCl 10 the spatial
L'ariable x.
PROOF OF PROPOSITION 30.10. As in the proof of Proposition 26.12, for each
t e ]0, T[, there exists an operator A(t): V ... V* such that
(A (I)"', v>v = a(t; '''', v) for all (7, M' e J':
By assumptions (A2 (A3), and the proof of Proposition 26.12. the assumptions
regarding A(t) in Theorem 30.A arc fulfilled.
As in Example 23.4, because fe Lq(Qr) there results the existence of a
function b e Lq(O, T; V*) such that
(b(t), v)y = b(t; v) for all v e  t E ]0, T[.
It follows from Section 30.1 b that problem (35) is identical to (4 and hence
(35) is equivalent to (1). 0
CoroUa!)' 30.12. Let X = L,(O, T; V). By Theorem 30.A, the generalized
problem (35) ;s equivalent to ti,e operator equation
u' + Au = b, u E W,.l(O. T; V, H),
u(O) = "0'
,,'here tI,e operator A: X ... X* ;s monotone, hemicontinuous, coerci'e, and
bounded.

30.S. The Main Theom on Semibounded Nonlinear Evolution Equations
783
In particular, all the results stated above hold true for problem (34) in
Example 30.8. In the special case of Example 30.8. the operator A: X ..... X. is
also 1I11iformly nWlwlolJe. In fact, by Proposition 26.10, we have
a(u.u - v) - a(vtll - v)  ellu - vll for all u, v E V,
and thus
(Au - Av. u - v)x = f: (A (I)U(t) - A (I)V(I). u(t) - v(t» dt
= foT a(lI(t), u(t) - v(t» - a(V(I). u(t) - vCr)) dt
> foT CUII(t) - v(t)lIdt
= c II u - v II  for all u, v EX.
30.5. The Main Theorem on Semibounded
Nonlinear Evolution Equations
We \\'ant to study the nonlinear evolution equation
u'(t) + A(u(t), t) = 0
for all I E [0, T],
(37)
u(O) = 1'0 e H,
where 0 < T < 00. Parallel to (37) we consider the problem
d
dt (u(t)IV)H + (A(u(t), t), v) = 0 on [0. T]
for all v e V, and u(O) = Ilo.
The point is that the operator
A: H x [0, To] -+ V+
satisfies the senliboundedness condition
(37*)
(A(I" t), v) > -c(llvll)
(38)
for all (v, t) e V x [0, To]. With respect to applications to nonlinear partial
differential equations, it is important that we use three different B-spaccs J':
H, V+ with
v s H s V+.
In order to obtain a great flexibility in applications, we do not assume that
V + is the dual space V* to J': We only assume that {J': V+} forms a dual pair
in the sense of Section 27.6. The following Theorem 30.8 is closely related to

784
30. Fil$t-Order Evolution Equations and the Galerkin Method
Theorem 27.B (the main theorem on locally coercive operators), where we also
made use of dual pairs. Recall that dual pairs also play an important role in
formulating general Fredholm alternatives. This can be found in the Appendix
to Part I (see A I (53». The simple idea of proof of Theorem 30.B below is to
use a Galerkin method for (37) in the "very nice" space V. The point is that
\VC obtain a priori estimates for the Galerkin equations only in the knice" space
H. Thus, a subsequence ('4,,) of the Galerkin solutions converges weakly in H
and the properties of A imply that the sequence (A (II,,(t). I» converges weakly
in the ubad" space V. .
Definition 30.13. We understand an adn.issible triplet U V c H c V+.. to be the
follo\ving:
(i) H is a real separable H-space with scalar product (.1. )H.
(ii) {Jt: V+} is a dual pair of real separable B-spaces with the corresponding
bilinear form <., · ).
(iii) The embcddings V s; H  V. are continuous and dense.
(iv) For all 11 e H, v E V,
(II, v) = (hlv)H.
(39)
EXAMPI..H 30.14. Each evolution triple is an admissible triplet. This (0110\\'5
from Problem 24.1.
Let {V, V+} be a dual pair. By definition, the \\.'eak+ convergence
+ . . n V -
".en  \v as n -. 00
means
("tn' V) -. <\V, v) aS'1 -+ XJ for all v E J':
If V" is equal to the dual space V* of V, then weak+ convergence is identical
to \veak* convergence. The \veak+ limit on V'" is unique, since (\t... v) = 0
for all v e V implies '" = o.
Definition JO.15. The function u: [0, T] -. V'" has the \veak+ -derit:ative
u'(s) = \". at the point s iff
u(s + II) - II(S) +
 W as', -. o.
h
For s = 0 and s = T, this is to be understood as a one-sided derivative.
This is equivalent to
d
dl (U(I). v> I,.,s :::: (w. v>
Let Y be a B-space. Then C w ( [0, T], Y) denotes the set of all functions
II: [0, T] -. Y. which are \veakl)1 continuous, i.e.. t..... ()'*, U(l) is continuous
on [0. T] for all }'. e Y*.
for all v e V.

30.5. The tain Theorem on Semiboundcd Nonlinear Evolution Equations 785
We make the following assumptions:
(H I) "V s H  y+ n is an admissible triplet.
(H2) For fixed To > 0, the operator A: H x [0, To] -. V+ is weakly
sequentially continuous, i.e.,
U.U in H
and
tIt -+ t in [0, To]
as n -+ 00
implies
A(rl", tn) A(u, t) in V+ as n -. 00.
(H3) Thc operator A is semiboululed, i.e., there exists a monotone increasing
C 1 -function c: R+ -+ R. such that the ke}7 condition (38) abo\'c holds
true.
(H4) Let U o E H be given.
Theorem 30.8 (Kato and Lai (1984). Assume (HI) throllgh (H4). 1"laelt there is
aT> 0 sflcl, that the original ilJitial value problem (37) has a solutioll u \\'ith
U € C w ( [0, T], H),
u' E Cw([O, T], V+),
(40)
",here u' denotes a ,,'eak. -derit'l{ltive. j\1oreot'er we hat'e u(t) -+ Uo in H as
I -. + o.
Al the same ti",e, II is also a solrltion of (37*).
CoroUa!). 30.16 (Majorization). For fixed TI e ]0, To], let g: [0, T.] ..... R+ be
a solrltion of the ordinar}' differential equation
g'(I) = 2c(g(t») OIl [0, T 1 ],
g(O) = 11110 II.
(41)
Tllen Theoren, 30.8 holds ,,'itll T = T 1 and "'e obtain
Ifr4(')I1 s g(l) on [0, T]
(42)
for tile solrltion II of (37).
We now consider the reversible case, i.e., we assume that the operator A in
(37) does not depend on time I and the semiboundcdncss condition (38) is
replaced with the follo\\'ing stronger condition:
I<Av, v)1 < c(l: t'II) for all v E  (43)
More precisely, we replace (H 1 )-(H4) with the follo\\'ing assumptions.
(H I.) .. V c II c V" ", is an admissible triplet.
(H2*) The operator A: H --t V + is weakly sequentially continuous and
continuous.
(H3*) There exists a monotone increasing C 1 -function c: IR+ -+ R+ such that
the ke}1 condition (43) above holds truc.
(H4*) Uniqueness. For each U o E Hit there is a To > 0 depending on Uo such
that problem (31) has at most one solution u with (40) and T = To.

786
30. First-Order Evolution Equations and the Galcrkin Method
Furthermore, we assume that the same uniqueness assumption is
valid for the modified problem (37) which is obtained from (37) by
replacing the operator A with - A.
Corollary 30.17 (Uniqueness and Regularity of the Solution). Assume (H 1*)
through (H4*). Let "0 e H be given. Then there is aT> 0 such tllat the original
;'J;t;al value problem (37) hCls €I unique solution
UE C1([0, T], V+)nC([O, T],H).
Notc that this is a much stronger result than Theorem 30.8. In particular,
we obtain that the problem
u' + Au = 0 on [0, T],
11(0) = Uo E H,
has a unique classical solution, i.e. t the derivativc u'(t) exists in the usual
classical sense on [0, T] with respect to the convergence on the space V.,. and
both u': [0, T] -+ V+ and u: [0. T] -+ H are continuous.
In the next section we \\'ill apply Corollary 30.17 to the generalized
Korteweg-de Vries equation.
The following proof of Theorem 30.8 is much simpler than the proof of
Theorem 30.A, since we no\\' have more regularity of the operator A at hand.
In addition, note that the scmiboundedness condition (38) is more flexible
than the global monotonicity condition for A in Theorem 30.A.
PROOF OF THEOREt.f 30.8. We set (ulv) = (ulv)H and 111411 = lIulin.
Step 1: Projection operator Pn.
We choose a sequence VI s; V 2 c: ... C V of finite-dimensional subspaccs
of V so that UtI  is dense in V. Then UtI  is also dense in H. Let {e 1 . . . . . ell}
be an orthonormal basis in  with respect to ( · ,.). We set
,.
P"II = L (u,e;)e,
'=1
for all 14 E V + .
Then the operator Pit: V. -+ V is linear and continuous, and we have
II
P,.II = L (ulei)ei
i-I
for all U ell.
i.e., P,.: H -+  is an orthogonal projection operator onto . Obviously, we
have
(v, P"u) = (II, P"v) for all 14, l' E V...,
P"II = u for all u E .
Slep 2: Solution of the Galerkin equations.
We consider the Galerkin equation
11(t) + P"A(ulI(t), I) = 0 on [0. T],
14,,(0) = P"uo.
(44)
(45)

30.5. The Main Theorem on Semiboundcd Nonlinear Evolution Equations
787
We seek a solution u,,: [0, T]   of this ordinary dilTerential equation
for suitable T > 0 independent of n. Since dim  < 00, the operator
P,.A:  x [0, 10]   is continuous. By the Peano theorem (Theorem 3.B)
there is a T* > 0 and a C 1 .s01ution II" of (45) on [0, 1'*].
Step 3: A priori estimates for the Galerkin solutions.
Our goal is to obtain the a priori estimates (47) below by using the majorant
equation (46b) below. From (44) and (45) we obtain
I d
2 dt " u ,,(t)1I 2 = (ulI(t)lu(t» = - (U,,(t). P.A(u,,(t), t»
= - <A(II,,(I) t), U,,(t»  c( lIu,,(t)n 2 ),
and lIu,,(O)1I = IIP"uoll  lIuoli. Thus, if u. is a solution of (45), then
d
dt II u,,(r) 11 2 < 2c( II U,,(t) 11 2 ), II U,,(O) 11 2 < II U o 11 2 , (46a)
and
d
de get) = 2c(g(I)),
g(O) = II Uo 11 2 .
(46b)
This implies the basic a priori estimates
lIu,,(t)H 2  get) on [0, T]
for all n.
(47)
More precisely, we have the following situation. If we extend the given
function c: IR+ --. R. to a monotone increasing CI-function c: R -. R, then it
follo\vs from the Picard-Lindelof theorem (Proposition 1.8) that there is a
TI > 0 such that the differential equation (46b) has a unique solution 9 on
[0, ]. Let t......llu,,(t)1I 2 be a solution of (46a) on [0, T], where 0 < T  T I .
Dy using a standard argument on differential inequalities (cf. Proposition
33.11), we obtain (47) from (46a) and (46b).
By the a priori estimate (47) and by the continuation principle (cf. Problem
30.2), we can extend the solution u.. in Step 2 to the interval [0, T 1 ]. The point
is that Tl does not depend on n. In what follows we set T = TJ.
Step 4: Convergence of the Galerkin method.
Let D be a dense countable set in [0, T]. By (47) there exists a constant C
such that
lIu,,(I)1I  C
for all 1 e [0, T]
and all n.
(48)
The H-space H is renexive. Using a diagonal procedure we can find a
subsequence, again denoted by (u,,), such that
U,,(l) u(t) in H as n -+ 00 for all lED. (49)
Let (e J) for fixed k and let n > k. By (44) and (45),
d
dt (u,,(t)lv) = - (v, P.A(un(t), t»
= - <A(U,,(I t v).

788
30. First-Order Evolution Equations and the Galerkin Method
Integration yields the ke}' relation
(u,,(t)lv) - (uolv) = - f (A(u,,(s).s).v) ds (50)
for all (' E  and all n ;> k. Note that (u,,(O)lv) = (P"uolv) = (uolv).
(I) We want to show that
u,,(t) 14(t) in H as n -+ 00 for all I E [0, T). (51)
The operator A: H x [0. T] -+ V+ is weakly sequentially continuous.
Since H is reflexive, A is bounded. Hence there is a constant K(v)
such that, for each v E Jt:
'(A(u,,(r), t v>1  K(v) (52)
holds for aliI e [0. T) and all II. We set /,,(1) = (u,,(I)lv) for fixed v e .
By (50) and (52 the sequence {J;.} is equicontinuous on [0, T].
According to Problem 19.14c, the limit
lim (u,,(I)lv) = f(t)
(53)
.'X)
exists for aliI E [0, T] and all v e lIt. This is true for all k, i.e., for all
(' E UA Jtt. Moreover, since UA:  is dense in Hand lIu,,(t)U S C, the
limit (53) exists for all v e Jt: This is (51).
(II) We show that
(u(t)lv) - (uolv) = - f (A (u(s), s). v) ds (54)
for all t e [0, T], t e J': In fact, for all (u, v) e V+ x Jt: we have
I(u, v)1 < constllullv.llvllv.
I t follows that u  < u, v) is a linear continuous functional on V" for all
v E  Relation (51) implies
A (U,,(I), I) -.'). A (u(t), t) in V+ as II -. 00
and hence
(A(un(t), t), v) -+ (A (u(t), r), t.') as n -. oc,.
for all f E [0, "r], v e V. Letting n --+ 00. equation (50) implies (54) for all
v e Uk . Since Uk  is dense in V and the bilinear form <.,.) is
continuous on V'" x V, we obtain that (54) holds for all v E V.
(III) I:rom (47) and (51) it follows that
Uu(t)1I < lim lIu,,(t)N S g(t)ll2 on [0, T] (55)
" -. QO
and hence lIu(t)1I  const on [0, T].
(IV) Weak continuity of u(.). Equation (54) sho\\'s that t t-+ (u(l)lv) is COD-

30.5. The Main Theorem on Semiboundcd Nonlinear Evolution Equations
789
tinuous on [0, T] for all veil: Since V is dense in Hand lIu(t)1I  const
on [0. T]. we obtain that 1'-' (u(I)1 v) is continuous on [0, T] for all
v e H, i.c., u e Cw([O, T], H).
(V) Weak to -differentiability of II. From (54) \\'e obtain
d
dt (u(t)lv) = -(A(u(t),t),v) on [0, T]
for all v e It: i.e., there exists the \vcak + -derivative
Ul(l) = - A (U(l), l) on [0, T].
(VI) Weak continuity ofu'.lf t -. son [0, T], then r4(1) u(s) in H and hence
A(U(l)tt)A(u(s),s) in V+; therefore, u' e C",([O. T]. V+).
(VII) Equation (54) shows that (u(O)lv) = (uolv) for all veil: Since Y is dense
in H, we get u(O) = 140'
(VIII) From
r4(t)uO in H as t -. +0
and II u(t) II 2  g(l) with g(O) = 11140112 we obtain
lIuoll S; lim lIu(I)1I < lim Ilu(t)1I < [Iuoll.
r+O .+o
This implies
Jlu(t)1I -. lIuoll
as t -. +0.
Since H is an H-space, this yields u(t) -. Uo in H as I  +0. 0
Corollary 30.16 follo\\'s from (55).
PROOF OF COROLLARY 30.17.
(I) By Theorem 30.8 and the uniqueness assumption (H4*), problem (37)
has a unique solution u E Cw([O, T], H) with the weak+ -derivative
14' e C.([O, TJ, Y+). Furthermore, we have
u(t) -+ u(O) in H as '''' +0.
(II) Letting v(t) = u(t + to) for fixed '0 e [0, T[, v is a solution of (37) \\'ith
v(O) = u(t o ). This solution is unique. Appl}'ing (I) to V t it follows that
v(t)  v(O) in H as t -. +0, and hence
u(t) -. U(lo) in H as I -. lo + 0 for each to e [0, T[.
(III) Letting '\'(1) = u( - t + T), ", is a solution of
",' - A", = 0 on [0, T], \",(0) = u(T). (56)
By (H4*), this solution is unique. Assumption (H3*) implies that the key
condition (38) holds for both the operators A and - A. Therefore, we can
apply the argument (I), (II) to equation (56). Hence
u(t)  rl(t o ) in H as l -. to - 0 for each Co e ]0. T].

790
JO. first-Order Evolution Equations and tM Galerkin Method
(IV) To finish the proof note the following. By (1)-(111), the function
u: [0, T] -+ H is continuous, i.e., u e C([O, T], II). Since A: H -t V+ is
continuous, the function t H Au(t) belongs to C([O, T], V+). Thcreforc,
noting (39), it follows from (54) that
u(r) - Uo = - f Au(s)ds for all r e [0. T].
This implics u'(t) = - Au(t) for all t e [0, T], and hcnce u' e C([O, T], V+).
o
30.6. Application to the Generalized
Korteweg-de Vries Equation
We want to apply the results of Section 30.5 to the following initial valuc
problem for the generalized Korteweg-de Vries equation:
II, + u,,,,,, + a(u)u,x = 0, x E R, t > 0,
u(x,O) = uo(x).
We are looking for the function u = u(x, t). Introducing the operator
(57.)
Au = U xxx + a(u)u x .
problem (57*) can be written in the form
U'(I) + Au(t) = 0, I > O.
u e H 3 ,
(57)
u(O) = 1'0.
For simplifying notation, we set
H'" = WieR), m = 0, 1, ... .
In particular, H O = L 2 (R). In the following, we will consider the case "0 e H Ift ,
m > 3.
Proposition 30.18. Suppose that the function a: R  R is C 3 . Let "0 e H 3 be
given. Then there is aT> 0 sue/. that the initial value problem (57) has a unique
so/llt;on
u e C 1 ([O, T],HO)nC([O, T],H 3 ).
The proof will be given below. This is a very natural result. since we shall
show below that Au is well defined for u e H 3 , i.e., the function u has general-
ized derivatives up to order three with respect to the spatial coordinate x.
Then Au e HO. More precisely, our proof will show that the operator
A: H J -+ H O

30.6. Application to the Generalized Kortewcg-dc Vries Equation
791
is continuous (and also weakly sequentially continuous). Therefore, by (57),
we expect that if 11o E H3 then U(I) E H 3 and hence u'(t) e HO for aliI e [0, T],
since Au(t) e HO. Indeed, Proposition 30.18 justifies this formal consideration.
The following stronger result shows that the solution u(t), t > 0, has the
same regularity (in terms of the Sobolev spaces H m , m > 3) as the initial value
u(O) = uo.
Proposition 30.19 (Regularity). Suppose that a: R -t> R is C. Let U o e II'" be
given for fixed In  3. Tllen tllere exists aT> 0 only depending on the norm
lIuoll H2 su('h that the initial value problem (57) MS a IIIlique soilltion
u e C 1 ([O, T], HM-3) n C([O, T], HM).
III addition, the Solulion u at lime t > 0 depends continuous/}' on the initial
talue Uo at time I = 0 ,,'itla respect to the Sobolev space Hilt. JWore precise I}', the
map
"0 ...... U
is cOIJtinuous fronl each ball B in H- into the space C([O, T], Hilt), ,,,here T
depends on B.
The continuous dependence of the solution" on the initial value U o can also
be expressed as follows. Let (uo,,) be a sequence of initial values such that
"0" "o in H-
as n ..... 00
for fixed In  3. Then there is an "0 and aT> 0 such that, for all n  no, the
corresponding solutions of problem (57) satisfy the condition
U..(I) -+ U(l) in H'" as n -t 00,
uniformly with respect to all t e [0, T].
Corollary 30.20 (C-Solutions). Suppose that a: Ii ... R ;s C. Let "0 be given
such that
Uo E C(R) " H'" for all m = 3, 4. . . . . (58)
e.g., Uo E CO(IR). Then the solution u from Proposition 30.19 satisfies
u(t) e (R)
for all t e [0, T].
PROOF OF COROLLARY 30.20. From (58) it follows that Uo E H'" for all m  3.
By Proposition 30.19. U(I) e Hili for all t e [0, T] and all m  3. By the Sobolev
embedding theorem, Hili  C"'-I(R) for all m  1. Hence u(t) e C(R). 0
Proposition 30.21 (Global Solutions for Small Initial Values). Suppose tllar
a: R ..... R is CtX'. TheIl tlrere is a number}' > 0 depending only on a( · ) such that,
for give" Uo e H'" ,vith fixed m > 3 and
II u ° II H:t < I"

792
30. First.()rder Evolution Equations and the Galcrkin Method
the initial f,"Qlue problenJ (57) has a unique solution
II e C 1 ([O, 00[, H-- 3) n C([O, 00[, H Ift ).
The following global existence theorem holds for arbitrarily large initial
values 110 in the case where the function a( · ) satisfies the following condition
lim 1).1- 6 f. ,l (l - p)a(p)dp S O. (59)
IAI-.x- 0
Theorem JO.C (Global Solutions of the Generalized Korteweg-de Vries Equa-
tion). Sllppose tllat a: R .... R ;s  alul suppose that cOlulitiolJ (59) 'Jolds. Let
110 E Hili be given for fi.'ted m  J. Then the original initial value problem (57)
has a unique solut ion
U E C 1 ([0, 00[,8"'-3) n C([O, 00(, H Ift ).
Remark 30.22 (Special Korteweg-de Vries Equation and the Method of the
Inverse Spectral Transform). In the special case
a(u) = -6u, (60)
the original equation (57*) is called the (special) Korteweg-de Vries equation.
The physical meaning of this important equation and its history will be
discussed in Chapter 71 in connection with nonlinear wave processes (cf.
Problem 71.7). Note that all the preceding results are valid for the special
Korteweg-de Vries equation, since condition (59) is fulfilled for (60).
For the special Korteweg-de Vries equation (57*) with (60), it is possible
to construct solutions of the initial value problem in an explicit manner by
using the method of inverse spectral transform. This method was discovered
by Gardner, Green, Kruskal, and Miura (1967). In fact. this was an important
discovery in modern mathematical physics. Before this discovery, mathe-
maticians and physicists believed that it would be extremely difficult to
construct explicit solutions for nonlinear evolution equations. Nowadays, we
know a number of imponant nonlinear evolution equations in physics which
allow the construction of explicit solutions via the inverse spectral transform.
This will be discussed in Problem 30.7. The method of inverse spectral trans-
form represents a sophisticated nonlinear variant of the classical Fourier
transform.
However. note that. in contrast to our general results above, the method of
inverse spectral transform is only applicable to equation (57*) in the case
where the function a( · ) has a special form.
PROOF OF PROPOSITION 30.18. We will use Theorem 30.8 and Corollary 30.17.
Step 1: Formal motivation of the choice of the spaces V s; H s V+.
Let u be a sufficiently smooth solution of the original problem (57.). that

30.6. Application to the Generalized Korteweg -de Vries Equation
793
is, u = u(x, t) and
II, + II""" + a(u)II" = 0, x E R, t > Ot
u(x.O) = uo(x).
Our goal is to write this in the form (37*), that is,
d
dl (u(l)1 v)" + (AU(l). v) = 0 on [0. T]
u(O) = Uo.
(61)
for all v E V,
(62)
To this end t \\'e set
v = H 6 , H = H 3 , V+ = HO.
Recall that Bm = W 2 "'(R) and HO = L 2 (R). Let ve Co(R). Multiplying (61) by
both v and -06V/OX 6 and using subsequent integration by parts. we obtain
(62) from (61) in the case where we set
All = 1I""x + a(u)u xt
(w. t') = fA ( WV - w :: )dX for all v E Y. \V E Y+. (63)
(ulv)" = fR (uv + uzzzvJCJCJC)dx for all u. t' e H.
In the following we set a"ujext. = u(t) and fR = J.
Step 2: Properties of the spaces H m .
Recall that
lIull"... = (f J lu(J)1 2 dx )'12, m = 0, 1, .. .,
and note that Co(R) is dense in H"'. m  O. The easiest way to investigate the
spaces H'" is to use the Fourier transform as in Section 21.20. For example, jf
Ii denotes the Fourier transform of u, then
flu(t'12dX = flta()12d (64)
for all U E q'(IR) and k = 0, I, .... In particular it follows from 112 <
const(1 + 114) for all  E R that
f U,2 dx < const f (u 2 + u"2)dx for all U e Co(R). (65)
(I) Equivalent scalar product on the space H. Analogously to (65), it follows
from (64) and
lIulii = (ulu)8 = f (u 1 + u"'1)dx

794
30. ....irst-Order E\'olulion Equations and the Galerkin Method
that
lIullH) < const lIuliH
for aU u e Co(R).
(66)
Since q(R) is dense in H = H 3 , relation (66) also holds true for all u e H.
Therefore, the space H is an H-space with respect to the scalar product
(III V)H introduced in (63
(II) Sobolev embedding theorem. Let C:(R) denote the B-space of all (;A-
functions ": R -+ R with
"
UuUcrc d.!.r > sup Iu4Jt(.)1 < 00
{:o xc:R
for fixed k = 0, I, ... . By Proposition 21.70, it follows via Fourier trans-
form that the embedding
Hili c C:- 1 (R), nl = 1, 2, ...,
is continuous.
In particular, since the embedding H 5 C;(R) is continuous, we get
lIu(t)lI c < constllul]H for all u e H, k = 0, 1, 2. (67)
Step 3: We show that V c H C Y. is an admissible triplet.
Using integration by parts, it follows from (63) that
("', v) = (\VIV)H for all "', v e Co(R).
Since Co(R) is dense in both H and V..., we obtain the compatibility condition
("', v) = (\"'lv)H for all \It' e H, v E V. (68)
In addition, we have to show that {V, V+} represents a dual pair with respect
to <., .). In fact, for all '" e V+, v e  we obtain from (63), by the Holder
inequality, that
1<"', v>12 < f ",2 dx f (v 2 + Iv(6)1 2 )dx
 II '" II  - II v f1  ·
Furthermore let v e V be given such that
< \to" v) = 0 for all w e V + .
By (68), (VIV)H = 0, and hence v = o.
Finally, let ".. E V+ be given such that
<\V,v) = 0
for aU v E 
We have to show that ,to' = O. To this end, we consider the equation
V o - V6) = "', Vo e  (69)
Recall that Y"" = L 2 (R) and Y = W 2 6 (R). It follows from the regularity theory
for elliptic equations that problem (69) has a solution Vo (cf. Problem 83.1).

30.6, Application to the Generalized Korte\\'eg-de Vries Equation
795
This implies
o = <"" vo> = f w 2 dx,
and hence '" = o.
Step 4: The operator A: H -. V. is continuous and weakly sequentially
continuous.
In fac it is obvious that the operator
u ..... ,,'w
is linear and continuous from H = H 3 into V+ = HO, since u"mll uo S lIull'l)
for all II e H 3 . By Proposition 21.81, each linear continuous operator between
B-spaces is also weakly continuous. In addition, it follows from Proposition
21.84 via Moser-type calculus that the operator
u t-+ a(u)u'
is continuous and weakly sequentially continuous from H into V. .
Step 5: We prove the following key condition.
There exists a monotone increasing C1.function c: IR+ -+ R. such that
I (Av, v)1 < c( IIvUl,)
To prove this, we set
(A v, v) = B(v, v) + C(v, v) + D(v, v),
for all v e 
(70)
where
B(v, v) = f (vNlv - v"'v(6t)dx.
C(v, v) = f a(v)v'vdx, D(v, v) = - f a(v)v'v( 6t dx.
Recall that H = H 3 . Thus, in order to obtain (70), we have to reduce B, C, D
to terms which only contain derivatives up to order three. This will be done
by using integration by parts.
(I) The first trick. Integration by parts yields
f vlllvdx = - f vv lll dx for all ve q(R)
and hence B(v, v) = - B(v, v) for all v e CO(R). This implies
B(v, v) = 0 for all v e J1:
(II) The second trick. Note that
VllYt'- =   V"'2. (71)

796
30. First-Order Evolution Equations and the Galerkin Method
Integration by parts yields
D(v, v) = J (a(tl)v')'" v'" dx
for all v E CO'(R).
By (71),
(a(v)v')Nl v tW = [a (t,)Nt v' + 3a(v)N VII + 3a(v)' v lN ] vlN + 2 -1 a(v)(v m2 )'. (72)
(III) The third trick. Let b: R -+ R be continuous. Then there exists a mono-
tone increasing Cl.function d: R ..... R such that
Ib(v)1 < d(lvl)
Therefore, for all v e C,,(IR
sup Ib(v(x»1  d( Uvllc).
.YeA
for all v e R.
Moreover, for all t,' e J': we obtain
sup Ib(v(x»1  d(K II vII " ),
xcR
(73)
where K denotes a constant. In this connection, observe that the em-
beddings V c H £ Cb(R) are continuous.
(IV) Proof of (70). Inequality (70) now follows easily from the tricks (1)-(111)
above and from the So bole\' embedding theorem (67). For example, for
all t,' e CO(R), we get
J a(v) (1,J»,z )' dx = - J a (v)' V""2 dx
- J a'(v)v'v"",z dx  d(KllvIl H ) J v'v.wz dx
 d(K II vII H) II v' lie II vII  S const d(K II vII " ) 11 vII .
In this connection, we use integration by parts as well as (67) and (73).
Similarly, using (72) we obtain
ID(v,v) + C(v,v)1 :s; c(lIv!l) (74)
for all v e C6(1R), where c: R. -+ R+ is an appropriate monotone increas.
ing C'.function. Since Co(R) is dense in II: a simple limiting argument
shows that (74) remains true for all v E V. This finishes the proof of (70).
Step 6: Existence.
It follo\\'s from Theorem 30.8 that, for given "0 E H. there is aT> 0 such
that the original problem (57) has a solution
u e C([O. 1'], H),
u' e C([O, T]. V+),
(75)
where the derivati\'e 11'(1) is to be understood in the sense of weak" conver-

30.6. Application to the Generalized Korteweg-dc \'ries Equation
797
gence, i.e., for all t' e II:
/ U(I + I,) - U(I) ) < ' ( \ , ) I 0
\ h ' v - U I"" as" - ·
From (69) it follows that, for each element \\. in V" = L 2 (R), there is a V o
in V such that J U\\' d., = J u(v o - V6) dx, i.e..
(ul "r)y. = (u, Vo > for all u e V....
Therefore. weak + convergence and weak convergence coincide on the space
V+. Hence the derivative '1'(1) also exists in the sense of weak convergence
on V....
Step 7: Uniqueness.
We show that the solution U of problem (57) is unique in the sense of Step 6.
(I) Let Uo e H be given, and let II and v be solutions of (57) such that 14 and
v satisfy condition (75). By Step 6. the derivatives u'(t) and v'(t) exist on
[0, T] in the sense of weak convergence on V.... According to Problem
30.9. this implies the continuity of 14, v: [0. T] -t V+ and
d
dl II "(1) - v(I)II. = 2("'(1) - v'(I)lu(l) - v(r)) on [0, T), (76)
\\'here ('1 .) denotes the scalar product on V+ = L 2 (R), i.e.,
("1 v) = fUVdX forall U,t'EV+.
From u' + Au = 0 and v' + Av = 0 we get
d
dt IIU(I) - v(t) II . = - 2(Au(t) - Av(t)1 u(t) - vet» (77)
for all t e [0, T]. We shall show below that there is a constant C > 0
such that
I(Au(t) - Ar;(t)lu(t) - v(t))1 S Cllu(t) - v(t)II. (78)
for all t E [0, T]. From (77) and (78) we obtain
lIu(t) - v(t)II.  lIu(O) - v(O)II. + f 2Cllu(s) - v(s)U:. ds
for aU I E [0. T]. Note that the integration of equation (77) is allowed,
since the right-hand side of (76), (77) is continuous on [0, T], by (75).
From the Gron\valllemnlQ in Section 3.5, we obtain
lIu(l) - v(t)1I V.  I! u(O) - v(O) II v. eCT
for all t E [0, T].
Since u(O) = v(O), this implies the desired relation
U(l) = v(t) for all I e [0, T].

798
30. First.()rdcr Evolution Equations and the Galerkin fethod
(II) Proof of (78). By (75), the functions u, v: [0, T] -+ H are weakly COD-
tinuous. Thus, we get
sup IIU(I)IIH + IIV(t)UH < 00
OS'T
(cf. Problem 30.9c). Let B be a closed ball in H. In order to prove (78) it
is sufficient to show that
I(AI4 - Avlu - v)1 < ellu - vll. for aU u, v E B, (79)
\vhere C depends on B. In what follows, note that the continuity of the
embedding H 5 C (iii) implies that
sup I",(.,)I + Iw'(x)1 s; constllwn" for all WE H. (80)
ER
Let u, V E CO(R) n B. Since Au = uIN + a(u)u', we have the decom-
position
(Au - Avlu - v) = (u D ' - v'»lu - v)
+ (a(u)(u' - v')lu - v) + «a(u) - a(v»v'lu - v).
(II-I) By the first trick from Step 5, integration by parts yields
(u Nt - v'"lu - v) = o.
(11-2) From
2a(u)(u' - v')(u - v) = «14 - V)2 a (U»' - (u - V)2 a '(II)U'
and (80) we get
12(a(u)(u' - v')lu - v)1
- 2 I a(u)(u' - v')(u - v)dx
- I a'(u)u'(u - v)2 dx
S const I (u - V)2 dx = constUu - vll..
(11-3) By the mean value theorem, there is a f) e [0, I] such that
a(u(x» - a(v(x» = a'(u(x) + .9(v(x) - u(x») [u(x) - v(x)],
and hence it follows from (80) that
IHa(u) - a(v))v'lu - v)1 = I (a(u) - a(v))v'(u - v)dx
< const IIU - vl 2 dx = constllu - vII;..

Problems
799
Therefore, relation (79) holds for all u, v E Ct(lR) " B. Since Co(R) is
dense in the spaces Hand V+, and since the operator A: H --. V. is
continuous, relation (79) also holds for all u, v e B.
Step 8: By Step 7, the same uniqueness result holds if we replace A by - A.
Consequently, Proposition 30.18 follows from Corollary 30.17. 0
Remark 30.23. In order to give an elegant proof of Propositions 30.19-30.21
and Theorem 30.C, we need nonlinear interpolation theory which \vill be
considered in Chapter 83 (cf. also Problem 30.11). We therefore postpone the
corresponding proofs to Chapter 83. At this place, we only mention the
following ideas of proof:
(i) The weak sequential continuity of the operator A: H'" --. H rn - 3 .. m > 3..
follo\\'s from Section 21.23 (Moser-type calculus).
(ii) The continuous dependence of the solution u on the initial values 1'0
follo\\'s from nonlinear interpolation theory which ensures the continuity
of appropriate nonlinear operators on interpolation spaces.
(iii) We use the method of norm compression in order to obtain the existence
of the solution on an interval [0, T] being independent of the space H"...
III > 3.
(iv) As usual, the existence of global solutions follows from suitable a priori
estimates.
PROBLafS
30.1. Proof of ln'nuJ 30.2.
Solution: We "'ant to use the substitution theorem A 2 (12). Let WE V be
fixed. We set
/(1, v) :: (A(t)v. "'>
for all t E ]0. T[. I' E II:
By assumption (H5) in Section 30.2, for each ve V. the function
t .-. /(1, v)
is measurable on ]0. T[. Moreover, for each t E ]0, T[, the function
v.-.+ f(t, v)
is continuous on V. since the operator A(t): V -+ V. is monotone and hemi-
continuous and hence demicontinuous.
Now let u e lp(O, T; V) be given, 1 < P < 00. Then the function t  u(t) is
measurable on ]0, T[. Therefore, it follows from A 2 (12) that the function
t t-t f(t. U(I)
is measurable on ]0, T[.
30.2. Continuation of the solutions of o,dinar)' differential equations in B-spaces. We

800
JO. First-Order Evolution Equations and the Galcrkin Method
consider the initial value problem
U'(I) = F(U(I), t) on J)
u(t o ) = Uo.
"'here J is a real finite or infinite interval ,,'ith to E J. i.e., J s R) and the map
F is of the form
(81)
F: X x J  X,
where X is a B-spacc. Let Uo E X be given. Suppose that the following a priori
esti ma te holds:
There is a po.itit.e number c such that if u: J o  X is Q solution of (81) on an
arbitrary subinterval J o of J, then
11u(I)1I :$; c on J o .
(82)
Show that problem (81) has a solution on J in the case where one of the
following four conditions is met:
(i) F is C. and bounded on bounded sets.
(ii) F is continuous. bounded on bounded sets.. and locally Lipschitz con-
tinuous with respect to u, i.e., for each (u, t) E X x J. there is a neighbor-
hood U (u, t) in X x J and a number L such that
II F(t,) s) - F(w, s)1I  L Dv - "'II
for all (v. s). (w, s) E U.
(iii) F is compact (e.g.. F is continuous on X x R and dim X < (0).
(iv) Let X = R. v and F = (F.,..., F N )) and let J be closed (e.g... J = R). There
is a real integrable function / e L. (J) such that
II F(u. t)1I S, /(I)
for all (u, t) e B x J,
(83)
"'here B = {u E X: lIuli  2c}. Moreover, F satisfies the Carathcodory
condition on 8 x J, i.e.. for all i,
t.-. (u. t) is measurable on J
u t-t fi(u. t) is continuous on B
for all U E B;
for almost all I e J.
In the cases (i) or (ii). the solution of(81) is unique on J.
In the case (iv), a solution is understood to be a continuous function
u: J  X such that the differential equation (81) holds for almost allt E J.
RenlQrk. In order to obtain a priori estimates of the form (82) one can use
integral inequalities (the Gronwall lemma in Section 3.5 or the gencrctli7.ed
Gronwall lemma in Section 7.3). as \\'ell as differential inequalities (see Proposi-
tions 33.11, 33.13, and 33.1 S).
Solution: We use similar arguments as in Section 3.3.
Slep I: Let J be a finite interval, e.g., let J = [to, t I]'
Ad(i). (ii). By Theorem 3.A, there exists aT> 0 such that equation (81) has
a unique solution on [to, to + T].
Let J. := [to, '.[ be the maximal half-open subinterval of J such that a solu-
tion u = u(t)or(81)exists on J.. The definition or J. makes sense.. since the solu-
tion of(81) is locally unique, by Theorem 3.A) and hence also globally unique.

Problems
801
Integrating equation (81). we obtain that
u(t) - u(s) = J: F(u(t). t)df
for all '0  s < 1 < '.. Since F is bounded on bounded sets,
M  sup . F(u, 1)11 < 00.
1_'  2('.IC J
(84)
B)' the a prior; estimate (82), we obtain that
jU(I) - u(s)1I  f M dt ... (I - s)M,
and hence the limit U(I) .... ". exists as t -+ '. - O. This implies t. = t 1. Other-
,,"isc. we could solve locall)' the new initial value problem
U'(t) = F(u(t), I),
U(I.) == u.'
by using Theorem 3.A. This way. we could continue the soJution u = U(I) of
(81) to a larger interval than J., which contradicts the maximality of J..
Ad(iii). By Theorem 3.B, there is a number T > 0 such that the equation
(81) has a solution u = u(t) on [to.'o + T]. Note the important fact that T
depends on/}' on the numbers c. J"" and t I - to. Hencc. the solution u = u(t)
can be continued to the interval J Iii: [1 0 , f 1] after a finite number of steps.
Ad(i\' We change F outside of the set B x J in such a way that condition
(iv) holds for B = X. To this end. we set
F(u, I) 1:1 F(u/2c( ull, t)
if lIuli > 2c.
No\\' apply the theorem of Caratheodory, A 2 (61), to the changed equation
corresponding to (81). Because of the a priori estimate (82) and the construction
of the set B, the solutions of the changed equation corresponding to (81) arc
also solutions of the original equation (81).
Step 2: Let J be an infinite interval, e.g., let J = R.
Let T> 0 be fixed. By Step 1. we can construct a solution u = U(l) or(81)
on [ - T, T], and we can continue this solution successively to the intervals
[ - n 1: n T], where n = 2, 3, . . . .
30.3. Proof of Lemnw 30.4. Use Problem 30.2 to show that the Galerkin equation
(5) has a unique solution for each n e N.
Solution: By (6), the Galerkin equation (5) represents a system of ordinary
differential equations.
The uniqueness of the solution follows from Section 30.3a. The existence of
a solution of(5) on [0, T] follows from Problem 30.2(iv) above. The assump-
tions (H 1)-(H5) of Thcorem 30.A imply condition (iv) of Problem 30.2. In this
connection, note the following. From (21) we obtain the a priori estimate
I u,,(I)1 < const on [0. T]
for all n.
B)' (H2), the operator A(I): V .-. V. is demicontinuous, and hence the function
(u, I)...... (A (t ) u, "i)
satisfies the Caratheodory condition on H,. x [0, T], according to (H5 Finally,

802
30. First-Order Evolution Equations and the Galerkin Method
from (H4) y,'c obtain the estimate
I<A(t)u. ";>1  const(c3(1) + lIuD"'.)U"J' (85)
for all (u, t) e H. x [0. 7.] and j = 1. . . . . n.
By the Caratheodory theorem. A2(61 the solution u M : [0. 1.]  Hit of (5) is
continuous. and the derivative u(t) exists for almost alii e [0. T]. Hence
u. E L,,(O, T; II,,).
8)' (85) and CJ e L.,(O. T the function
t t-+ <A(l)UM(t "i>
belongs to Lf(O, T). Moreover, it follows from I(b(t). "i>r S Bb(t)W'II\\jIlO and
b € L.(O. T; V*) that the function
t  <b(t "J>
also belongs to L.(O, T). Finally. by the Galerkin equation (Sa), we get
u e Lo(O. T; H.).
30.4. Proof of Lemma 30.7.
Solution: We will use a similar argument as in the proof of Theorem 23.A.
(I) Let II E W"I (0, T: Jt: H) be the solution of the original problem (J), and Jet
u" be thc solution of the Galerkin equation (5). By Step 5 of the proof of
Theorem 23.A. thcrc cxists a sequence (p,,) of polynomials PIt: [0. T] -+ Hit
such that
P. -+ u in lit (0, T;  H)
as n -t X) t
and hence
Pn -+ U in C([O, T], H)
as n -t 00.
(86)
By Lemma 30.5, u,,(O) -+ u(O) in H as n ... 00, and hence
P.(O) - u,,(O) ... 0 in H
Below we shall show that
max lu.(t) - p..(l)l;, - lu,,(O) - p,,(O)I  0
Ors T
as n -+ 00.
(87)
as n  x,. (88)
This )'ields the desired result, namely,
u"  u in C([O, T], H)
as n  00,
by (86) and (87).
(II) We prove (88). From the original problem (1),
u'(t) + A(t)u(I) - bet) = 0,
and from the Galerkin equation (5),
<U(l) + A(I)U,,(I) - b(t). t:) = 0
for all v e H".
wc obtain
(u(t). u,,(t) - P.(l) > = (b(t) - A(I)U,,(I), u.(t) - p,,(t»
= (U'(I) + A (t)u(t) - A(I)U,,(I), U..(l) - p,,(t».

Problems
803
B)' the monotonicity of A(t).
(A(t)u(t) - A(t)u.(t). u,.(l) - u(t» < O.
Finally, for allt e [0, T], the integration by parts formula (10) yields the
following ke}' relation:
lUu,,(t) - p.(t)l - lu,,(O) - p.(O)I)
"" L (u(s) - p(s), u,,(s) - p,,(s» ds
.. L (u' + Au - Au" - p,(u. - u) + (u - p.»ds
 J (u' - p;, u. - PIt) + (Au - Au", u - p,,)ds
< lIu' - plIx.llun - p.D x + fiAu - Au.Dx.llu - p.n x c1d d..
Since u" -+ u and Pn -. u in X as ,. -. 00, the sequences (u.) and (p,,) are
bounded in X. Because A: X --. X. is bounded, the sequence (Au,,) is
bounded in X.. Therefore, we obtain that
d" S const.: u - p.II... -+ 0
This implies (88).
30.S. The spectral transform. The follo\\'ing considerations will be used basically in
Problem JO.7. The connection with scattering theory in quantum mechanics
win be explained in Problem 30.8 below.
We consider the stational)' SchrOdinger equation
as n -+ 00.
- .p1¥(X) + u(x)"'(x) = k 2 .p(x).
(89)
We assume that the real CrrJ.potential u vanishes sufficiently fast at infinity,
i.e.,
J: lu(x)l(1 + Ixl)dx < 00.
We are looking for complex-valued eisenfunctions.p. The spectrum of(89) has
the foJlowing structure:
(i) Continuous spectrum. For each real k :;: O. the number k 2 is a double
eigenvalue of (89) with the two linearly independent eigenfunctions '" I
and "2' which are uniquely characterized by the following asymptotic
behavior:
.pI (x) = e- uu : + 0(1),
tP2(X) = eatA' + 0(1),
Additionally, we obtain
.p I (_) = a(k)e -;1.& + b(k)e l1JC + o( 1).
.p2(X) = b(J()e- iAz + a(Ij e ilz + 0(1).
x  -00
(90)
x ... -.
X -+ +cx;.,
(91)
X -+ +aJ.

804
30. First-Order E\'olution Equations and the Galerkin Method
The matrix
T ( a(k) b(k» )
= oro Q(1(J
(92)
is called the transition matrix.
(ii) Discrete spectrum. Equation (89) has either no negative eigenvalues or a
finite number of negativc eigenvalues
-00 < kf < ki < ... < k. < o.
All these eigenvalues are simple. Letting k j = iqJ with qJ > O. the cor-
responding eigenfunctions t/lUJ,j = 1,..., N, are uniquely charactcrized by
the following asymptotic behavior:
\fI(j)(x) = e flJ1l + o(e. r ), x -+ -co. (93)
Additionally.. we have
.p<JJ(x) :;; cJe-flJ:l + o(e-4tz),
x -. +oc.
where fJ is real.
The mapping
u..... (a(k), b(k}. qj. Cj) (94)
with k € Rand j ::: I,..., N. is called the spectra/transform.
In terms of quantum mechanics, (i) and (ii) above correspond to scattered
panicles and to bound states of particles. respectively. In the special case
u(x) 5! 0, there are no negative eigenvalues. and (90) holds with 0(1) = O.
30.6. The int"erse spectral transform. Let all the scattering data a(k). b(k). qJ and
ci be given. We want to construct the corresponding potential 21. To this end.
we set
F  cJe- flJ 1C 1 J  b(k) ;( dk
(x) = I.- + - - e
ja-I ;a'(iqj) 2n -CZJ a(k) ,
and we consider the so.callcd Gelfmld-Let.';talJ-Marcenko equation
K(x,,.) + F(x + y) + J....: K(x,z)F(z + y)dz = O.
If we know a solution K of this linear integral equation. then wc obtain the
unknown potential u by the relation
d
u(x) = -2 dx K(x, x).
The dctails can be found in Novikov (1980. M) and Levitan (1984, M).
30.7. COIJstructioIJ of solutions of the KorteM"eg-de Vries equation via the inverse
spectral transform. We consider the nonlinear Korteweg-de Vries equation
21, + U:l - 6uu 1C =: 0,
-ct:J < x < 00.. t > 0..
(95)
U(X,O) = uo(x)
together \\'ith the linear SchrOdinger equation
- \lI"(,) + u(x. t)f/I(x) = 0
(95.)

Problems
80S
for fixed, but otherwise arbitrary I. Then we have the following important
result:
(R) Let u = u(x t) be a soluI;on of (95), which t'Qnishes sufficiently fast as
Ixl -+ a:,1. Then the speclrallran.yorm of u satisfies the following t1i!ry simple
linear equation:
d(kt I) = 0.
q} = O.
b(k, t) = 8ik J b(kt t
c} = 8qJC J I
(96)
The dot denotes the I-deri\'ativc.
According to (R), we can use the following elegant procedure in order to
solve the initial value problem (95).
(i) For given initial values u = uo(x). \\"C compute the spectral transform
a(k, O b(k, 0). qj(O). Cj(O).
(ii) We solve equation (96). This yields
a(k, t) = a(k,O). qj.l) = qJ(O)t t > 0.
b(k, t) = b(k,O)eBfi:a,. cJ(t) = cO)eS4J(O)r.
(iii) We get the solution u = u(x. I) of the original problem (95) by using the
inverse spectral transform
(a(k, I) b(k, l) qJI), CJI).... u(x t I),
which we considered in Problem 30.6.
30.7a. J\Olt)l;oot;on of (R). In order to display the simple idea of proof as clearly as
possiblc. we restrict ourselves to formal considerations.
(I) Discrete spectrum. We introduce the following two differential operators:
d 2
L(I)'" = - dx .p(x) + u(x t I).,(X
d) d c
A(I) = 4- d 3 tjI(x) - 3u(x,t)- d \If(x) - -;-(u(x. I)t/I(X».
x x vX
where the fixed function u satisfies the Korteweg-de Vries equation (95).
From (9S we get the key relation
L. = LA - AL.
(97)
Hence
1..(1) = e - AI L(O)e A '.
(97.)
The unitary group {eA'} is to be understood in the sense of a ske\\'-adjoint
extension of the skew-symmetric operator A in L(R) with D(A) = Ct(A).
By (97-), the operator L(t) has the same eigenvalues as L(O) i.e., we obtain
the crucial relation
qJ(I) = qJ(O)
for all t,
and hence 4) := O. The pair {L, A} \\'ith (97) is called a La. pair. Lct
'" =- t/J(x t) and let
L(I)-It = - q2tJ1
(98)
for fixed t, where q = qJ- By Problem 30.5 for fixed I, the eigenfunction .p

806
30. First.Order Evolution F..qualions and the Galerkin Method
can be characterized by the folloy,'ing asymptotic behavior:
(x, t) -= e t1l + o(e fZ ),
.. .... -00.
(99a)
In addi tion,
.;(x, t) = c(t)e- fJC + o(e- 4X ),
X -+ +00.
(99b)
Differentiation of (98) with respect to time t yields
L,e/! + Le/!, = _q2t/1,.
Notethatq ;;: qJisindependentofl. By (97) and (98).(L + q2)(tIt, + At/!) = O.
Hence we obtain
L(1)q> = _q2tp,
where qJ = tjI. + Ae/!. By (99a).
(f) = 4q 3 e 4 .1 + o(e), x -+ -aJ.
Hence tp ::: 4q" e/!. i.e.. we obtain the key equation
"', + At/! = 4q" t/!.
(100)
In this connection, note that u is rapidly vanishing as Ixl ... 00, i.e., we may
put A = 4d J /dx 3 as Ixl-+ OC). Finally, it follows from (99b) and (100) that
e(l) = Sq3C(I
(II) Continuous spectrum. Let k 2: 0 be fixed and let t/1 denote the eigenfunc-
tion of the equation
L(t)t/1 = k 2 .;
(101)
for fixed t, where t/! = "'(x, t) is characterized by the following asymptotic
behavior:
"'(x, t) II: e - .1e1l + o( 1),
x -. -00.
(102a)
I n addition,
ap(x, I) = a(k, t)e-. ax + b('" t)ef"X + o( 1),
X -+ +00. (I02b)
As above. differentiation of(101) with respect to time t yields
L(t)q> = k 2 cp,
where cp = "', + Ae/!. By (102a).
tp -= 4ik 3 e-u;z + o( I), x -+ -00.
Hence tp .. 41k 3 t/1, i.e., we obtain the key equation
"', + At/! = 4ik 3 e/!.
( 103 )
Using (102b) we get
. d 3
de-tAx + be ib s (-4- + 4ik J )(ae-£t1l + be tAx ) + 0(1)
dx 3
= Sik 3 be ib + 0(1). x -+ +00.
Hence d  0 and b = 8ik 3 b. This finishes the motivation of (R).
For more details compare Novikov (1980, M) and Levitan (1984. M).

Problems
807
30.7b. Discussion of the method oJ' the int'erse spectral transform (.9').
(i) Explicit solutions. The method (.<7) reduces the integration of a nonlinear
partial differential equation to the integration of linear ordinary differential
equations. In a certain sense, one obtains the solutions in an explicit manner
in contrast to general existence proofs via the Galerkin method. There exists
a number of important nonlinear evolution equation in physics. which can
be treated similarly. A list of 37 equations which can be solved by the in-
verse spectral transform method, can be found in Calogero and Degasperis
(1982, M). Compare also Mihailov, Sabat and Jamilov (1987). We also recom-
mend Bullough and Caudrey (1980. S), Novikov (1980. M Ablowitz and Segur
(1981. M), Faddee\' and Takhtadjan (1987, M).
(ii) Nonlinear Fourier transform. The method (,Y') represents a nonlinear
\'ariant of the classical f"ourier transform. To explain this, consider the linear-
ized Korteweg-de Vries equation
u, + u xJCx =: O.
Using the Fourier transform
u(x, t):: Joo b(k. t)e ib dk,
\\'e get
J: (6 - iP b)e 1iX dk = O.
This implies ;, ::;; ikJb and hence
b(k, t) = ea, b(k, 0).
(iii) IlJfinite-dimens;onal integrable Hamillonian sySlem. The Hamiltonian
system
, oH(p, q)
Pi = - ,
oqJ
4 oH(p.q)
J- ,
oPJ
j= I....,N..
( 104)
is called integrable if there exists a sufficiently regular transformation P &I
P(p.q). Q ;; Q(P.q) such that (104) is transformed into the simple equation
=
aR(P)
oQJ ·
A _ aR(p)
'lJ - op.. '
J
j= I....,N.
(105)
Equation (105) has the solution
aH(p)
fj(t) = const. QJ(t) = 0 t + const. (106)

Transforming back to the variables q and p, we obtain the solution of (104).
In terms of classical mechanics. the Hamiltonian H frequently represents
the energy of the system where q) and Pj correspond to a position coordinate
and a generalized momentum, respectively.
Note the crucial fact that . j = 1..... N. are conserved quantities by (106
i.e..  is constant along each solution of(I04). Moreover. H is also a conserved

808
30. First-Order Evolution Equations and the GaJerkin Method
quantity (conservation of energy). In fact, it follo\\'s from (104) that
 H(p(l), q(t)) = H,p + H.4 = qp - pq = 0
for aliI.
The Korte\\'eg-de Vries equation (95) and equation (96) correspond to (104)
and (IOS respectively. Moreover, the canonical transformation P :I P(p,q
Q a= Q(p. q) corresponds to the spectral transform. Morc precisely, set
H(u) = I-: (r l u: + uJ)dx. (107)
For all h E Co(R), we obtain the first variation
H'(u)h =  H(u + lh)/.=o
= f>o (u"h" + 3u 2 h)dx = f (3,,2 - uu)hdx.
We define the variational derivative H/bu(x) by the relation
f a: bH
H'(u)/a = .. hdx
-2' bu(.)
for all h e C:(R).
Hence H/bl'(.) = 3u 2 - UJrJt' Thus. the Kortcy,'eg-de Vries equation u, +
U'''fX - 6uu,K -= 0 ma}' be written as the following infinite-dimensional Hamil-
tonian system
C bll
u, :;II - .
ex 6u(x)
\\'hich corresponds to (104). The important Hamiltonian approach to
nonlinear e\'olution equations can be found in Faddccv and Takhtadjan
(1987, M). Physicists like to use the Hamiltonian approach in order to
get maximal insight and to find the quantized form of ph)'sical theories
(cf. Part V),
(iv) Conservation of energ}' and an infinite number of consert'Qlion laws.
As we mentioned above, the function 1/ from (104) represents the energ}'
of systems in classical mechanics.. In Problem 71.7 we shall sho\\' that the
Korteweg-de Vries equation (95) describes the motion of an infinite number
of nonlinearly coupled oscillators (nonlinear oscillations of a system with an
infinite number of degrees of freedom). We therefore expect that II(u) from
(107) represents the energy of the oscillating system which is a conserved
quantit}, (conservation of energy). More precisely, we shall sho\\' the follo\\'ing.
If I'  u(.., I) is a solution of the Korteweg-dc Vries equation (95), \\'here
u e C 4 (LQ2) and u( " I) e Co (R) for alii E R, then
H(U(I)) = const for all t e [R.
(107*)
The same remains true if, for each fixed I, Ihe function U  u(x.t) and its
x-derivatives vanish sufficiently rapidly as Ixl -. 00.
In fact, we obtain from (95) that u, = 6uu z - Uza.x and U z . = 611; + 6uu.r.x -

Problems
809
U XKXX . Hence
d J 2;
d - H(I,(r» = (uxu.u + 3u 2 u,)dx
t -/rl
J 2: V
= -;-(6uu; - 3u 2 u xx - !uxx + Ju 2 )dx = O.
- t71 (.i X
More generall)" one can show that there exists an infinite number of conserved
quantities for the Kortewcg-de Vries equation. Roughly speaking, this reneets
the fact that (95) can be regarded as an integrable Hamiltonian system with
an infinite number of degrees of freedom. Recall that the classical system (104),
y,'ith N degrees of freedom, has the N conser\'ed quantities , j == I,..., N.
Generally. conservation laws play an imponant role in the theory of non-
linear evolution equations, since the)' represent a priori estimates for the
solutions. which are necessary for proving global existence (cf. Chapter 83).
(v) SolitolJs. The simple spectral transform
b(k,O) = 0,
k -;q
a(k.O) = k . ' q, C
, + Iq
corresponds to the solution
24 2
I'(X, t) = -
cosh 2 q(x - 4q 2 , - 2)
of the Korteweg-de Vries equation (95), where  = (ll2q) In c (cr. Novikov
(1980, M»). This solution is called a solitary wave or a soliton. Note that the
shape of this propagating y,'ave remains constant for all times t (Fig. 30,1).
(vi) Collision betMleen solitons. Using the spectral transform
N k - iqJ
b(k.O) = O. a(k.O) = n k . ' qj. c j .
J-t + IqJ
one can construct solutions of the Korteweg-de Vries equation which cor-
respond to N solitons (cf, Novikov (1980. M)). It is remarkable that such
solitons behave elastically in collisions, i.e., collisions between solitons do ,.ot
change the speed and the shape of the solitons (Fi 30.2). This behavior of
solitons is vel)' interesting from the physical point of \'iew, Roughly speaking.
in many fields of physics, solitons describe the strictly localized transport of
energy, "'here the transported energy behaves like a particle, This represents
a basic phenomenon in natufC. This is why ph)'sicists arc very interested in
the theory of solitons..
The method of inverse spectral transform goes back to Gardner, Green,
KruskaJ, and Miura (1967). This method represents an important discovery
in mathematical physics. More about the interesting histor)' of this method
can be found in Problem 71.7.
A general representation formula for explicit solutions of the Korteweg-de
Vries equation via theta functions and its discussion can be found in Its and
Matveev (1975), Mat\'ee\' (1986, S), Bobenko and Bordag (1987), and in the
monograph by Bobcnko, Its, and Matveev (1991). In this connection, it is quite

810
JO. First-Order Evolution Equations and the GaJerkin Method
u
.t

...
Figure 30.1


.
(a)

..

(b)
Figure 30.2
remarkable that the explicit solutions of nonlinear evolution equations are
parametrized. in some sense. by Riemannian surfaces. The theory of theta
functions can be found in Mumford (1983, L). Cf. also Toda (1981, M).
30.8. Scattering of parlicles in quantum mechanics and the ph}'s;cal background of
the spectral transform.
3O.8a. Classical mechanics. The equation
nix = - V'(x)
( I 08)
describes the motion x = X(l) of a particle of mass m on IR. Here V is ca1led a
potential. The momentum vector is given by p = nIXe, where e denotes the unit
vector in the direction of the x-axis. Equation (108) implies conservation of
energy, i.e.., for each solution of (108). there exists a constant E such that t for
all It
2
E =  + V(x(t)).
(109)
Here E is called the total energy. ConsequentlYt the motion of the particle is
only possible in such regions where x satisfies the condition
E - V(x)  o.
Figure 30.3(a) and (b) corresponds to a bounded and an unbounded motion.
respectively. In terms of our solar system, the motion of planets and comets
corresponds to bounded and unbounded motions. respectively.

Problems
811
E
/
v
. x
E
v
.t
(a)
(b)
Figure 30.3
JO.8b. Quantum mechanics. In terms of quantum mechanics. the motion of a particle
of mass m on R is described by the SchrOdinger equation
1J2
ih(/'r = - 2m (/'X1f + V (/', ( 11 0)
where" = h/27t, and h denotes the Planck quantum of action. Letting
ih
v ;::; 2 - (qJ<p - qJfI'll)e.
mp
P = f/Jt:p,
we obtain from (110) the continuity equation
P, + div pI' = O.
(111)
Each physical quantity in classical mechanics corresponds to an operator in
quantum mechanics. For example, energy E and momentum vector p corre-
spond to the following operators:
a
E - Ih at .
. " 0
p  -, e a .
This way, we obtain the Schrodinger equation (110) from the classical energy
equation (109) (quantization or classical mechanics).
(i) Bound states. Let (f' be a solution of (110) with II' E L(R) normalized by
r  p dx = I. Then. by definition. the number
i b pdx
is equal to the probabilit). for finding the panicle in the interval [a. b]. Such
so-called bound states correspond to bounded motions in classical mechanics.
(ii) (.inbound Slales. Let (f) be a solution of (110) with f p dx = ex;. Such
so-called unbound states correspond to unbounded motions in classical
mechanics. In this case, the function cp describes a stream of particles, \\'here
P represents the particle density (i.e.. the number of particles per volume) and
v represents the velocity vector. The continuity equation (Ill) above describes
the conservation of the number of particles (cr. Section 69.1).

812
30. FiBt-Order Evolution Equations and the Galerkin Method
Let'" be a nonzero solution of the stationary SchrOdinger equation
h 2
E'" = - 2m t/lJe,x + V';.
( 112)
\\'here E is a fixed real number. Then the function
cp(x, t) = e-'ErlJ\,,(x)
is a solution of the SchrOdinger equation (110). If J(1) 1';1 2 dx = 1, then tp
corresponds to a bound state of energy E.
I n contrast to this situation, the function
IJ 2 k 2
E= 2m .
satisfies the SchrOdingcr equation (110) with vanishing potential V :s O. Since
I 1(,01' J2 dx = 00. the function lp-r describes a stream of free particles with
particle densit)' p :E I cP 1: 1 2 = 1 and velocity vector
CwO %(x. t) = e-1£ItttetiAi..
hk
v = + -e.
m
The momentum vector p and the kinetic energy E tin of one particle are given
by the relations
P Ie mv a: + hke,
mv 2
E kiD =- - IiiiI E.
2
JO.8c. The spectral transform. By Problem 30.8b. the spectral transform
u...... (a(k), b(k), qJ. c j )
of Problem 3O.S allows the following physical interpretation:
(i) The function u in (89) corresponds to the potentia) V = IJ2 u{2m of the
Schrodingcr equation (112).
(ii) The value qJ corresponds to an eigenvalue E ;;; - qf h 2 12m of (112). i.e.,
qJ corresponds to a bound state of energ)' E.
(iii) The function tP. with the asymptotic behavior
tP.(x) = e-il:. + 0(1), x -. -00.
till (x) - a(k)e- iU + b(k)e ib + 0(1), x ... + XI.
corresponds to the scattering of a particle stream on the x-axis caused by the
potential  More precisely, the original particle stream a(k)e- ax at x - +0::
with velocity vector v = - hkelm splits into:
(a) the reflected particle stream b(k)e iAIr at x .. +00 with velocity vector
v = ',kejm and
(b) the transmitted particle stream e- ib at x = -00 with velocity vector
v = - hke/m (Fig. 30.4
Scattering theory in quantum physics will be studied in greater detail in
Pan V (ef. Chapters 89 and 92 on quantum mechanics and quantum field
theory. respectively).

Problems
813
<
reflection
/
/ ..
<:
..t
Figure 30.4
30.9. Weak deritl;t'eS. Let u: [0, T] -+ X be a given function, where X is a real
H-space and 0 < T < 00.
30.9a. For fixed I e [0. T]. suppose that the derivative u' (I) exists in the sense of weak
convergence on X, i.e.,
U(I + h) - U(I) ' ( ) . X
h u I In
as h -. O.
( 113)
Note that if I = 0 or I = 1: then this limit is to be understood as a one-sided
limit. Show that u is continuous at t and that
d
dt (u(t)lu(l)) = 2(u'(t)lu(t)).
Solution: By the uniform boundedness theorem AI(3S relation (113) implies
( 114)
U(t + II) - u(t) I
sup h < .
 Silo
Thus, " is continuous at I. Consequently, the sequence
(u(t + h)lu(t + h) - (u(t)lu(t» ( U(t + h) - u(t) h )
h = h U(I) + u(t + )
goes to 2(u'(t)lu(t» as h  O.
30.9b. Suppose that, for each t e [0. T], the derivative u'(t) exists in the sense of weak
convergence on X and u' E Cw([O. T], X i.e.. u': [0, T] ..... X is weakly con-
tinuous. Show that u: [0, T] ... X is Lipschitz continuous.
Solution: The set {u'(t t e [0, T]} is bounded in X, by Problem 30.9c.
For fixed v E X . let <.o(t) = (u(I)1 v). By the mean value theorem. <p(I) - q>(s) =
«p'()(l - s and hence
I(U(I)lv) - (u(s)lv)1 = l(u'()lv)(t - s)1
< ( sup IIU'()II ) IIvllll - sl.
oss T
This implies
lIu(l) - u(5)11 S constlt - sl
for all I. s e [0. T].

814
30. First-Order Evolution Equations and the Galcrkin Method
.. JO.9c. Let u: [0, T] -. X be weakly continuous. Show that
sup lIu(t); < 00.
OSI T
Solution: If the assertion is not true.. then there exists a sequence (I,,) in [0, T]
such that. u(t,,)11 -+ . as n -+ . Since [0, T] is compact. we may assume that
I" -t l as n -t a), and hence u(,.)u(') as n  00. Thus, (U(I,,)) is bounded in
X. This is a contradiction.
30.10. Approximalion method of Rothe. With this method, the time derivatives
of nonlinear parabolic differential equations are discretized. Study Kacur
(1985. M) and Schumann (1987). Cr. also Chapter 83.
30.11.. J\lonlinear interpolation theory and the continuit}' of nonlinear operators on
interpolation spaces. In the Appendix. A2(112 we summarize basic results of
linear interpolation theory. We want to generalize this to nonlinear operators.
To this end. we consider schematically the following situation:
X!;;y!;;Z
1 ! ! s ( II 5)
g s V s 2.
lt X, g and Z, 2 be real B-spaces. where the embeddings X s; Z and r s 2
arc continuous. For fixed 0 < 0 < I and I S P < 00, we set
y = [Z, X].., V = [2, 1],."
i.e., Y and Y arc interpolation spaces in the sense of the K-method (cf. A 2 (112)).
In applications. the space X is nicer than Z, i.e., the functions in X are smoother
than those in Z. etc. We are given the nonlinear operator
S: M  Z ....2, (116)
where J'" is an open subset of Y (e.g.. J\1 = Y). Show that S(J\f) S Y and that
S:MsYi' (117)
is continuous with respect to the nice spaces Y. V in the case where the follo\\'ing
two conditions arc satisfied:
(i) The operator S is Lipschitz continuous with respect to the '.bad" spaces
Z, 2. i.e.. there is a constant L > 0 such that
IISu - Svllz < Lnu - vllz for all u. ve M.
(ii) The operator S is bounded with respect to the "very nicc" spaces X, r, i.e.,
there is a constant C > 0 such that S(M " X) s; X and
IISu H, < C(I + lIux) for all u e M n X.
Hint: This is a special case or a more general result due to Gunther (1987),
which we will prove in Chapter 83.
Special rose of linear operators. Let the operators S: Z .-. 2 and S: X ..... X
be linear and continuous. Then conditions (i) and (ii) arc satisfied. Hence the
operator S: Y ... V is also linear and continuous.
Important applications to nonlinear evolulion equatiolLc;:
u'(t) + Bu(t) = O. 0 < r < T.
u(O) = u o .
( 118)

RcfcrenQC$ to the Literature
815
Such applications will be considered in Chapter 83. The main idea is the
following. Let u = U(I) be the unique solution of (118). We set
Suo = u(t)
for fixed time t. From our continuity result above we obtain that the solution
or( 118) depends continuously on the initial data. More precisely, we shall show
chat this continuous dependence is uniform with respect to all t e [0, T] in
the case where the constant C in (ii) above is independent of t (d. Chapter
83).
For example. in Chapter 83. we shall apply the above result to the general-
ized Kortey,'eg-de Vries equation by letting
X =r X =- W 2 "'(R),
z = 2 ;;I L1(R
and
y = Y = W 2 A (R).
I S k < m.
Here. condition (i) (i.e.. the Lipschitz continuity of the evolution operator S)
follows from the Gronwall lemma similarly as in the proof of Proposition
30.18, and condition (ii) (i.e., the boundedness of S) can be verified easily.
References to the Literature
Genera1 expositions: Lions (1969, M) (numerous methods). Caron (1969. M). Gajewski.
Grager, and Zacharias (1974, M Barbu (197 M Tanabe (1979. M), Henry (1981. L).
Haraux (1981, L), Visik and Fursikov (1981, M Pazy (1983. M Smoller (1983, M).
Majda (1984. L v. Wahl (1985. M), Browder (1986. p Pavel (1987. M BcniJan.
Crandall, and pazy (1989. M). Christodoulou and Klainerman (1990. M).
Survey articles: Dubinskii (1968 (1976). v. Wahl (19781 (19821 Kato (1986). Crandall
( 1986).
Scmibounded evolution equations and the Euler flow for incompressible inviscid
fluids: Kato and Lai (1984). Kato (1986. S).
Quasi-linear parabolic differential equations: Oleinik and KruZkov (1961, S). Visik
(1962), Ladyienskaja (1967. M, B. H). Friedman (1964. M). (1969. M1 Lions (1969. M).
Dubinskii (1968, S), (1976, S Amann (1986), (1986a-c1 (1988), (1988a-c).
Semilinear parabolic equations and reaction-diffusion processes: Henry (1981. L)
(recommended as an introduction), Fife (1979, L) (applications to mathematica1 biology
Smoller (1983, M), Rothe (1984, L), Amann (1984). (1985), (1986c). (1988), (1988a).
Browder (1986, P).
Semilinear evolution equations and existence and nonexistence or global solutions
in reactor dynamics: Pao (1978), ( 1919), (1980).
Pseudoparabolic equations: Showalter (1977, M) (linear equations (1978, M) (non-
Newtonian fluids), Gajewski, Groger, and Zacharias (1914, M Caroll and Showalter
(1976, M), &Ohm and Showalter (1985) (nonlinear diffusion), Kaur (1985, L) (Rothe.s
method for pseudoparabolic equations).
Scales of B-spaces, hard implicit function theorem, and evolution equations of the
Cauchy- Ko\'alevskaja type: Nirenberg (t 912), O\'sjannikov (1976) (applications to the
shallow water theory), Nishida (1971). Hamilton (1982. S). Deimling (1985, M). Walter
(1985).
The Korteweg-de Vries equation, solitons, and the inverse spectral transform:
Noviko\' (1980, M) and Ablowitz and Segur (1981. M, H) (recommended as an

816
30. First-Order Evolution Equations and the Galerkin Method
introduction), Gardner. Green. Kruskal. and Miura (1967) (classical paper), Lax (1968
Bullough and Caudrey (1980. P) (applications to physics), Calogero and Degasperis
(1982, M), Lee (1981. M) and Rajaraman (198 M) (solitons, instantons, and ele-
mentary particles). Davydov (1984. M) (solitons in molecular s)'stems), Knorrer (1986,
S) (solitons. integrable Hamiltonian systems. and algebraic geometry), Faddeev and
Takhtadjan (1987, M) (infinite-dimensional Hamiltonian systems, solitons, the
Riemann - Hilbert problem, and in\'ersc scattering theor)'), Bobenko and Bordag (1987)
(multi-cnoidal waves). Konopclchenko (1987, M) (nonlinear integrable systems in
physics), Ne\\'ell (1987, S).
Nonlinear lattices in physics: Toda (1981, M).
General solutions of the Kortcweg-dc Vries equation and of other nonlinear e\'olu-
tion equations \'ia multi-dimcnsional thcta functions and Riemannian surfaces: Its and
Mat\'ee\' (1975), Toda (1981, M), Matveev (1986, S), Bobenko and Bordag (1987).
Bobenko.lts. and Mat\.ccy (1991. M
Theory of theta functions: Mumford (1983, L).
Complete classification of integrable systems: Mihailo\', Sabat, and Jamilov (1987,
S).
Magnetic monopoles: Atiyah and Hitchin (1988, M).
Collection of classical papers on solitons: Rebbi (1984).
Scattering processes in quantum mechanics: Schechter (1981, M) (introduction),
Reed and Simon (1971, M). Vol. 3, Berezin and Shubin (1983, M)(cr. also the References
to the Litcrature for Chapter 89).
Inverse spectral theory: Poschel and Trubowitz (1987, M).
The gcncralizcd Konc\\'cg-de Vries equation: Bona and Smith (1975). Bona and
Scott (1976), Kato (1983, S). (1986), KruZkov and Faminskii (1985 Gunther (1987)
(applications of nonlinear interpolation theory; cf. Chapter 83).
JVun,er;cal,nethods
Handbook of numerical analysis: Ciarlet and Lions (1988, M  V ols. 1 fr.
Finite clements and the Galerkin method: Thomee (1984, L) (recommended as an
introduction Douglas and Dupont (1970, S), Glowinski, Lions, and Trcmolicrcs
(1976. M Ciarlet (1977, M Temam (1977, M), Fletcher (1984, M), Gekeler (1984, M
Reinhardt (1985. M Girault and Ra\'iart (1986, M).
Fractional step method: Raviart (1967), Janenko (1969, L), Temam (1968). (1977, M),
Lions (1969. M).
Regularization methods: Lions (1969, M
Approximation method of Rothe: Kacur (1985, M Schumann (1987).
Difference mcthods: Meis and Marcowitz (1978, M Marchuk and Shaidurov (1983,
M). Reinhardt (1985, M).
Multigrid methods: Hackbusch and Trottenberg (1982, P), Hackbusch (1985. M).
Supercomputer methods: Murman (1985, P), Lichnewsk)' and Saguez (1987. S
Martin (1988, S).
(Cf. also the References to the Literature for Chapters 31- 33.)

CHA PTER 31
Maximal Accretive Operators,
Nonlinear Nonexpansive Semigroups,
and First-Order Evolution Equations
The analytical theory of scmigroups is a recent addition to the ever-growing list
of mathematical disciplines .... I hail a semigroup when I see one and I
seem to see them everywhere! Friends have obsented., ho\\'e\'er, that there are
mathematical objects which arc not semigroups.
Einar Hille ( 1948)
The importance of the class of nonexpansive mappings lies neither in its trivial
generalization of a Lipschitz condition, nor in a comparable durability or
fruitfulness. but in two ke)' observations: first, nonexpansive mappings arc
intimately tied to the monotonicity methods developed since the earJy 1960's.
and constitute one of the first classes of mappings for \\'hich fixed-point results
""ere obtained by using the fine geometric structure of the underlying Banach
space instead of compactness properties. Second, they appear in applications as
the shirt operator for initial value problems of differential inclusions of the form
u'(I) + A(I)U(I) 30,
where the operators A(I) are in general multivalued. and in some sense positive
(accretive) and only minimally continuous.
Ronald Bruck (1983)
The condition of maximal accretivity is cnjo)'cd by man)' important operators
in applications.
Michael Crandall (1986)
In Chapter 30 we considered first-order evolution equations of the form
U'(I) + A(t)u(t) = b(l), u(O) = "0 E H, (I)
with the operators A(t): V -+ V. and bet) e V. for all t e ]0, T[. In this
connection, .. V c: H c: V." is an evolution triple. In this chapter. we
investigate problems of the form
u'(t) + Au(r) = 0, u(O) = Uo e H, (2)
817

818
31. Maximal Accretive Operators and Nonlinear Noncxpanslve Semigroups
with the operator A: D(A) S; 11 -+ H. Whereas we use the three spaces V. H,
V. in (I) in an essential way, we work in (2) only in the H-space H. However,
in this connection, A is not necessarily defined on the whole space H. The
approach in Chapter 30 was a generalization of the Galerkin method for linear
evolution equations in Chapter 23. In this chapter, we want to generalize the
theory of linear nonexpansive semigroups studied in Chapter 19 via the
Yosida approximation. In Theorem 19.E we showed that a linear densely
defined operator - A on an H-space is the generator of a linear nonexpansive
scmigroup iff A is maximal accretive. As we will show, the theory of nonlinear
nonexpansive semigroups on H-spaces and B-spaces is based on nonlinear
nJaximal accret;t'e operators. We suppose that A in (2) is maximal accretive.
Let u = U(l) be the unique solution of (2). If we set
U(I) = S(I)Uo. (3)
then {S(l)} is a nonlinear nonexpansive semigroup. In Chapter 55 (resp.
Chapter 57), we shall consider the more general problem
u'(t) + Au(t) 3 b(t),
u(O) = uo,
(4)
where A: X .... 2 x is a multivalued maximal accretive operator on the H-space
X (rcsp. B-space X). If b(t) = 0, then the solutions of (4) generate a non-
linear nonexpansive semigroup {S(t)} according to (3). Thus, in contrast to
linear nonexpansive semigroups, nonlinear nonexpansive semigroups can be
generated by multivalued operators. If A: D(A) e X..... X is single-valued
then we obtain the corresponding multivalued operator A: X -. 2 x by setting
Au = {Au} for u e D(A) and Au = 0 for u f D(A). Then (4) goes over into the
equation
14'(t) + Au(t) = b(t
u(O) = uo.
In an H-space one has the following fundamental equivalence:
(4*)
A is maximal accrelit e. A is maximal monotone.
This is the source for the close connection between nonexpansive semigroups,
first-order evolution equations, and the theory of monotone operators.
In this chapter the main idea is the following. Besides the original equation
u'(t) + AU(I) = 0, u(O) = uo
we consider the well-behaved approximate equation
u(t) + A"u,,(t) = 0, U,.(O) = U o ,
where All = A(l + IlA)-l is the Yosida approximation of the operator A. Since
A,. is Lipschitz continuous the approximate equation can easily be solved by
using the Picard-Lindelof theorem (Theorem 3.A). The point is to consider
the passage to the limit p ..... + O. In this connection, the maximal accretivity

31.1. The Main Theorem
819
of A plays a fundamental role. More precisely, we use the fact that the maximal
accretivity of A implies the maximal monotonicity of A. Hence we can apply
the trick of maximal monotonicity.
The same method can be used to prove the existence of solutions for
equation (4) in case X is an H-space (cf. Chapter 55). In Chapter 57, we will
use a completely new technique in order to obtain generalized solutions for
the general class (4) of multivalued evolution equations in B-spaces, where A
is maximal accretive.
31.1. The Main Theorem
We consider the equation
u"(t) + Au(t) = 0, 0 < t < 00,
u(O) = u o .
By definition, the derivative u'(t) exists in the sense of weak convergence iff
(5)
U(I + h) - U(I) ' ( ) ' H
h -:. u 1 In
If we replace un by "-.", then we obtain the derivative in the usual sense.
as h -+ O.
Theorem 31.A (Komura (1967). Let A: D(A) S H -. H be an operator on the
real H-space H K'ith the folloK'ing two properties:
(HI) A is Inol,otone, i.e., (Au - Avlu - v)  0 for all u, ve D(A).
(H2) R(l + A) = H.
Then, for each given Uo E D(A). there exists exactl)' one continuous function
u: [0, oo[ -. H such that equation (5) holds for all t E ]0,00[, ,,,here the
der;vat;f,'e u'(t) is to be understood in the sense of weak convergence.
J\{oreover, this uI,iqlle solution has the following additional properties:
(a) u(t) E D(A) for all t > O.
(b) u(.) is Lipschitz continuou. on [0, 00[.
(c) For almost all t e ]0, 00[, the derivatill'e u'(t) exists in the usual sense and
satisfies equation (5). Furthermore, we have Hu'(t)1!  IIAuoli.
(d) The function t.-. u'(t) is the generalized derivative of the function
t...... u(t) on ]0, 00[. Moreot'er, u" e C..([O, 00[, H), i.e., u'(.): [0, oo[ -. H is
K'eakl}' continuous.
(e) For all t  0, there exist the derivative u(t) from the righl and
u(t) + Au(t) == 0,
u(O) = Uo.
In the next section we will prove the following equivalences:
(H 1), (H2) <:> A is maximal accretive  A is maximal monotone.

820
31. Maximal Accretive Operators and Nonlinear Noncxpansi\'e Semigroups
Corollary 31.1 (Connection with Nonexpansivc Semigroups). Let u = u(t) be
the solution of equation (5). We set
5(1)110 = U(I) for all I  0 and r40 E D(A). (6)
Theil, {S(I)} is a nonexpans;ve sem;gro up 01 ' D(A) \\'I,;ch can be IIniquely
e.--:lended 10 a nonexpalasive se migr oup on D(A).
The gellerator of {S{t)} 011 D{A) ;s - A.
Definition 31.2. If we set u(t) = 5(1)140 for r40 E D(A), then u(.) is called the
generalized solution of (5).
II is possible to give a complete characterization of the class of all nonlinear
nonexpansi\'c scmigroups on H-spaces. To this end one needs equation (5),
where A is a multitalued maximal monotone operator. This will be considered
in Section 32.24.
31.2. Maximal Accretive Operators
In order to make the proof of Theorem 31.A, in the next section, as transparent
as possible, we first prove some results of general interest.
Definition 31.3. Let A: D(A) c H  H be an operator on the real H-space H.
(i) A is called monotone iff (Au - Avlu - v) > 0 for all u, v E D(A).
(ii) A is called ma,,-.;;mal monotone iff A is monotone and
(b - At'lu - v) > 0
for all v E D(A)
implies Au = b, i.e., A has no proper monotone extension.
(iii) A is called accret;L'e iff (I + pA): D(A) -+ H is injective and (1 + pA)-1 is
nonexpansive for all Ii > O.
(iv) A is called maximal accrelive(m-accretive) iff A is accretive and (1 + liA)-l
exists on H for all JJ > o.
For historical reasons, the use of the term "accretive" is not uniform in
the literaturc. We follow the modern theory of nonexpansive semigroups.
Maximal accretive operators are sometimes called "hypermaximal accretive"
or "hyperaccretive."
Proposition 31.4. Tile operator A: D(A) c H -t H on the real H-.pace H ;s
nlonotone iff it is accretive.
PROOF. For all u, f,' E D(A) and Jl > 0,
!I(u + pAu) - (v + pA (,) II 2 = lIu - ('11 2 + 2p(Ar4 - AI'lu - t')
+ Jl211Au - Av1)2.

31.2. Maximal Accreti\'c Operators
821
Hence \\'e have
n(u + pAu) - (I' + IIA I') II 2 > lIu - vII 2
iff (All - Avlu - v)  o.
for all J.l > 0
o
Proposition 31.5. Let A: D(A) c H .... H be an operator on the real H-space H.
TlJellthe follo",jng tlJree properties of A are mutrlall}, equivalent:
(i) A;s monotone a,ul R(l + A) :: H.
(ii) A is maximal accretit'e.
(iii) A is maxilnal Inonotone.
We prove (i) => (ii)  (iii). Notc that only these implications are needed in
the proof of Theorem 31.A below. The implication (iii) => (i) is a famous result
of Minty (1962) which stood at the very beginning of the modern theory of
monotone operators. In Section 32.4 we shall prove the main theorem on
multivalued maximal monotone operators in B-spaces via a Galerkin method.
Then the implication (iii)  (i) is a simple consequence of this main theorem
(cf. Proposition 32.8).
PROOF. (i)  (ii). This follows from the Banach fixed-point theorem. We give
the simple proof in Problem 31.1.
(ii)  (iii). Let A be maximal accretive. Then A is monotone by Proposition
31.4. Suppose that
(b - Avlll - v)  0 for all Ii E D(A). (7)
We set v, = (1 + A)-l(U + b + IZ) for t > 0 and z e H. Addition of (7) with
t' = v, and (II - v,lrl - v,)  0 yields
(b + u - Av, - v,lu - v,)  o.
Hence (zlu - v,) s O. Letting l ... 0 \ve obtain
(zlu - (1 + A)-l(b + u» S 0
i.e., u = (1 + A)-1 (b + rl). Hence Au = b.
for aU z e H,
o
Proposition 31.6 (Convergence Trick of Maximal Monotonicity). Let A:
D(A)  H .... H be maxinzal monotone on the real H-space H. TheIl, it /ollo,,'s
fron! either
Au b
"
as n.... 00,
u. .... u
as n -II 00,
or
Au" .... b
as n.... oc,
u u
"
as n.... 00,
that Arl = b.

822 31. Maximal Accretive Operators and Nonlinear Nonexpansive Scmigroups
PROOF. From (Au" - Avlu" - v)  0 for all n it follows that (b - Avlu - v) >
o for all t' E D(A). Hence Au = b. 0
31.3. Proof of the Main Theorem
31.3a. Proof of Uniqueness
Let II: [0, oo[ -+ H be a solution of (5), where u is continuous and U'(I) exists
for all t E ]O oo[ in the sense of weak convergence. By Problem 30.9,
d
dt IIII(t)1I 2 = 2(u'(t)lu(I»
for all t e ]0, 00 [.
Let v be another solution of (5). Then, for all t e ]0, 00[.
d
de lIu(t) - v(t)1I 2 = 2(u'(t) - v'(t)lu(t) - vel))
= - (AU(I) - Av(t)lu(l) - v(t) S 0,
since A is monotone. Hence
II U(/) - v(I)1I S lIu(O) - v(O)1I
for all t > o.
(8)
Therefore. since u(O) = v(O), we get lI(t) = vet) for all t  o.
31.3b. Proof of Existence
The following proof of Theorem 31.A generalizes the proof of Theorem 19.E.
Step 1: The nonlinear resolvent RIJ = (I + pA)-l for all p > o.
It follows from (H I), (H2) in Theorem 31.A and from Proposition 31.5 that
A is maximal accretivc. Hence the operator R" exists for allll > 0 and
R,,: H -+ D(A)
is bijective and nonexpansive, i.e.,
UR,.u - R,.vll s: lIu - vn for all u, veil, Jl > O. (9)
Step 2: The nonlinear Y osida approximation A,. = p-l (1 - R,.) for all J.l > o.
Lemma 31.7. For all p > 0 a,ul u, ve H ,ve have:
A"u = ARIJII,
ItA"u - Apvll S 2jJ- 1 1lu - vII.
(A"u - A...vlu - v)  O.
That means, AIJ is Lipschitz cont;n'lous and monotone.
(10)
(11)
(12)

31.3. Proof of the Main Theorem
823
For a/lp > 0 and u e D(A), we have R"Au = A"u and
IIA,.ull S IIAu[l.
(13)
PROOF. Equation (10) follows from A_R;1 = Jl-I(R;I - I) = A. Rclation (9)
implies that
IIA"u - A"vll = Jl- 1 11(u - v) - (Rllu - R,av)1I  2p- 1 1lu - vH.
IrA_ull = Jl-ll1u - R"ull = p-IIIR,,(1 + pA)u - R,.IIU  IIAIIII.
The operator R" is nonexpansive. Thus, 1 - RIJ is monotone (cf. Problem 31.3).
and hence A" is monotonc. 0
Step 3: The operator A: D( A) S X .... X with X = L 2 (O, T; H) for fixed
T> o.
We \\'ant to extend the operator A: D(A) c H -. H to the H-space X in a
natural way. To this end, we set
(AU)(I) = Au(t)
for almost all l e [0, T].
(14)
To be precise, we define the domain D(A) to be the set of all u e X with the
property that
14(1) e D(A)
holds for almost all t e [0, T] and t t-+ Au(t) belongs to X.
Lemma 31.8. The operalor A: D( A) s:; X -. X is maximal accretive and
maxinJal nJOIJOlolJe.
This follows from the maximal aecretiveness of A. We give the simple proof
in Problem 31.2.
Step 4: The solution of the auxiliary problem:
u(t) + A"u,,(t) = 0, 0 < I < 00.
u,,(O) = Uo e D(A).
The operator A" is Lipschitz continuous on H. By the global Picard-
Lindelof theorem (Corollary 3.8 problem (15) has exactly one C 1 -solution
II: R -. H.
Let u" and v" be two C1-solutions of(15). As in the uniqueness proof above,
it follows from the monotonicity of A" that
(15)
1Iu,,(t) - v,,(I)11  1111,,(0) - v,,(O)1I
Step S: Estimates for U".
for all I  o.
(16)
Lemma 31.9. For all I, S  0 and all  l > 0, the following Ilold:
IIU(l)1I = IIA"u,.(t)1I s IIAuolI,
IIU,.(I) - ulJ(s)1I < IIAuolllt - sl.
(17)
(18)

824 31. taximal Acxretive Operators and Nonlinear Noncxpansi\'c Scmigroups
lIu,,(I) - R"rl,.(t)1I  JlII Auoli.
II II" (t) - II A (t ) II < 2 J Jl + l (t II A Uo II ).
(19)
(20)
PROOF. Ad( 17). Along with t...... u,,(t), the function t ........1I,.(t + IJ) with II > 0 is
also a solution of(15) with appropriate initial values. It follows from (16) that
II u" ( l) - U II ( t + II) II  II U JI (0) - II ,. (h) II.
Dividing by " and then letting II --+ + O. we get, by (13), that
1111  (t) U S II I,; (0) II = ]I A" Uo II < I! A Uo tl.
Ad( 18). This follows from the mean value theorem (Proposition 3.5) and
( I 7).
Ad(19). Note that A" = p-l(1 - R p ) and use (17).
Ad(20). We set
A = -(A"U,.(I) - AA 11;.(t)IRAu,t(t) - U.t(I) + U..(I) - R..II,.(t).
By (17) and (19
I I < 2(p + iv) II Arloll z .
Taking into consideration the differential equation (15), All = AR". and the
monotonicity of A" we have that
I d
2 dt IIU,.(I) - U,t(I)U 2 = (U(l) - u(t)luJI(t) - u;.Ct))
= -(A"II,.(t) - AArl;.(t)lu,,(I) - U.t(I»
= -(AR,.u,,(l) - AR,tI';.(t)iR"u,,(t) - RAII).(t» + 
 .
Now. (20) results from this, after integration over [0, t]. In this connection,
note that U,.(O) - 11;.(0) = O. 0
Step 6: Convergence in H as Jl -+ + O.
By (20), 11,,(1) converges in H as J --. + 0 to a certain u(t) and indeed
uniformly \vith respect to all compact t-intervals. Inequality (18) yields
1111(1) - u(s)U < II AuoH It - sl for all 't S  o. (21)
Step 7: Convergence in X = Lz(O, 7.: H) as p  + O.
The uniform convergence in the preceding step yields
Il,.  II in X as J.l  +0.
By (21). the function l t-+ U(I) is Lipschitz continuous on R+. According to
Corollary 23.22, the derivative u'(t) exists for almost all I E R... Furthermore.
it follows, from (21). that
lIu'(t)il < IIAu o l1 for almost all t e R.t

31.3. Proof of the Main Theorem
825
i.e., u' E X. Moreover, u' is the generalized derivative of Il on each interval
]0, T[. By (17), there exists a constant c such that
lIu IIx s c for all Jl > O.
As an H-space, X is reflexive. Therefore, by passing to a suitable subsequence,
we obtain that
14" -t> U in X
and
uw in X
as JJ --. +0.
By Proposition 23.19, it follows that
,
u = \v.
Because of
U(l) = - AuJl(t) = - AR"u,,(t)
and (19), the follo\\'ing hold:
RII fl,. ..... fI in X
-AR U"t in X

as Jl-+ +0,
as Jl'" + o.
The operator A is maximal monotone. Hence the cOllvergellce trick of maxim'!..l
nlonotonicit)1 (Proposition 31.6) yields the fundamental result that u e D(A)
and
-
w = -Au,
i.e., u' = - Au . This implies u'(t) = - Au(t) for almost aliI e IR+.
Let t > O. From IIAR"u,,(t)1I < IIAuoU, by (17), and from (19) in connection
",.ith Step 6, it rollow for an appropriate subsequence, that
Ru,,(I) -+ U(I) in H as I'  +0,
ARfI,.(t)z in H as I' -+ +0.
Since A is maximal monotone, we obtain u(t) E D(A) and AfI(t} = z.
Consequently,
IIAu(t)1I < lim (lAR"u..(t)1( S IIAfloli.
,,-+0
If ,..... u(t) is a solution of (5), then t  rl(t + s) is also a solution of (5) with
initial value II(S). Hence
II Au(t)U :s; UAu(s)1I
for all t > S > 0,
i.c., the function t .-.IIAII(t)U is monotone decreasing on IR+.
Step 8: The function t Au(t) is continuous from the right on R+.
Let 'n \. t as n ..... 00. Then IIA(rl(tn))1I  IIAu(t)U for all n. Thus, there exists
a subsequence, again denoted by (AII{'n)), such that
Au(t,,)  z as n -+ 'X),
u(t,,) -. Il(t) as II -+ 00.

826 31. Maximal Accretive Operators and Nonlinear Noncxpansivc Semigroups
Since A is maximal monotone, Au(t) = z. The sequence (II Au(tn)lI) is monotone
decreasing and hence convergent. Furthermore, Au(t.) Au(t) as n  00
implies
JlAu(I)1I s lim II Au(t.)11  lJAu(t)lI,
...
and hence 'Au(',,)11 -. II Au(t)lI. Thus, Au(t.) -. Au(t) as n .... 00, by Proposition
21.23(d). The convergence principle (Proposition 10.13) implies Arl(s) -. Au(t)
as S -+ t + O.
Step 9: Derivative from the right.
Let h > o. Integration of u'(t) + Au(t) = 0 yields
u(t + h) - u(r) 1 f. '+11 ( ) d
h = - h Au s s.
,
By Step 8,
d"
di"u(t) = -Au(t)
Step 10: Weak continuity.
The same argument as in Step 8 shows that In -. t as n -+ 00 implies
Au(t,,)  AU(I) in H as n -. co, i.e., for each '" e H, the function
for all t  0.
t...... (Au(t)1 M')
is continuous on [0. 00[.
Step 11: Weak derivative.
In what follows let I. S  O. Jl > 0, and w e H. From (15) we get
(u,,(t)lw) = (uolw) - J (A,.u..(s)lw)ds.
By (17)
I(A,.u,.(s)lw)1 s IIAuolIlI\yll.
Noting that A,. = ARII' we obtain from Step 7 that
A,.u,.(r)....a.. Au(t) in H as II- -. + o.
By Step 6, 14,,(1) -. 14(1) in H as JJ ... + O. Therefore, as JJ ... + 0, majorized
convergence yields
(u(t)lw) = (uolw) - J (Au(s)lw)ds.
Since the integrand is continuous by Step 10, we obtain
I . (u(t + h)I\\l) - (u(t)I\\1) (A ( )) )
1m h = - u r w.
o
That means
14'(1) == - Au(t),

31.4. Application to Monotone Coercive Operators on B-Spaocs
827
\vhere the derivative u'(t) is to be understood in the sense of weak convergence
on H.
The proof of Theorem 31.A is complete. The proof of Corollary 31.1 will
be given in Problem 31.4. 0
31.4. Application to Monotone Coercive
Operators on B-Spaces
Proposition 31.10. Let A: D(A) c H -. H be a linear operator on tire real
H-space H. Then A ;s maximal monotone iff - A is maxinlal dissipative.
PROOF. This follows immediately from Definition 19.44 and Proposition 31.5.
o
We now want to explain the connection between maximal monotone
operators and the Friedrichs extension.
EXAMPLE 31.11. Let A: D(A) s; H  H be a linear symmetric and strongly
monotone operator on the real H-space H, i.e.,
(Aulu» clluIl 2 foral) ueD(A) and fixed c>O. (22)
Let A F : D(A F ) c: H -. H be the Friedrichs extension of A with the corre-
sponding energetic space HE. By Section 19.10, there exists an energetic
extension A E of A such that
A  A F c: A£
with D(A)  D(A F ) C H£ c H c Hl, and the operator
AI;: HE -+ Hl
is a linear, strongly monotone homeomorphism. Moreover, the Friedrichs
extension A F is the restriction of A E to D(A F ) = ASl(H). Then (22) also
holds for AF' and the operator A F is self-adjoint. By construction of AF'
R(l + A F ) = H. Hence A F is maximal monotone.
Consequently, the Friedriclls extension of A is a maximal monotone
extension of A.
Proposition 31.12. LeI A: D(A) s; H -. H be a linear symlnetric monotone
operator on the real H-space H, i.e.,
(Aulu) > 0
for all u E D(A).
Then A is maximal monotone iff ;t is self-adjoint.
PROOF. Let A be self-adjoint. By Problem 19.7, al11 < 0 belong to the resolvent
set of A. In particular, R(l + A) = H, i.e., A is maximal monotone.

828
31. Maximal Accretive Operaton and Nonlinear Noncxpansive Scmigroups
Let A be maximal monotonc. We set B = I + A. Then B is strongly
monotone. Let B F be the Friedrichs extension of B. Then A  B F - I. The
operator B F - I is self-adjoint. Since A is maximal monotone, there is no
proper monotone extcnsion of A. Hence A = B F - 1, i.e., A is self-adjoint. 0
We no\\' want to generalize the situation of the -riedrichs extension in
Example 31.11 to nonlinear operators. At the same time, we want to explain
the connection with evolution equations. To this end, we consider the two
evolution equations
u/(t) + A£U(I) = 0, 0 < t < 00,
u(O) = u o ,
"'here the derivative u'(t) is to be understood in the sense of the convergence
in H, for almost all t e ]0, oc [, and
(23)
u'(t) + Au(t) = 0, 0 < t < 00,
u(O) = u o .
Furthermore.. we consider the equation
(24)
d
dl (11(1)1 w) + a(u(I). w) = 0
forall ,,,,eH, 0<'< XJ,
11(0) = uo.
Here, we set a(lI, v) = (A£u, v).,.
We make the following assumptions:
(H I) ,. V c H £ V." is an evolution triplc. In particular, this means that V is
a B-space, H is an H-space, and
(25)
(II, v)., = (1JIv)n
for all hell, v e V.
(H2) The operator A E : V -. V* is monotone. hemicontinuous, and coercive.
By Theorem 26.A, the operator A E is surjective.
(H3) We set D(A) = {" e V: Au e H} and Au = AEu for all 14 e D(A).
Proposition 31.13. SI4ppose that (HI) through (H3) hold. TlJen:
(a) 1lIe operator A: D(A) c H -. H i. maxillJalllwIJotone.
(b) Eacll solution of equation (23) is also a SOI,l,;on of (24). f'or ever) U o e D(A).
equation (24) has a unique solution in the seIJse of Theorem 31.A. This
solutio'! is also a so lution of (25).
(c) For each 14 0 e D(A), equation (24) has the generalized solution rl(t) = 5(t)uo,
",/Jere {S(t)} ;s tile IJoIJexpansive senJigroup generated b} - A.
PROOF. The operator A£ is monotone, i.e.,
(A£u - A£v,u - v)y > 0
for all u, v E 

31.S. Application to Quasi-Linear Parabolic Differential F.quations
829
Since AEu = Au e H for all u e D(A)t we obtain
(Au - Avlu - v) > 0 for all u. I' ell.
Let I denote the identity operator on H. Then
«At + I)U,II)v = (A£u,u)., + (ulu)
for all u e H.
Thus, the operator A E + I: V -. V* is monotone, hemicontinuous, coercive,
and hence surjective by Theorem 26.A. This implies R(A + 1) = H, i.e., A is
maximal monotone.
Let u = u(t) be a solution of (23). Then u(t) e V i.c., U(I) e Hand u'(t) E H.
This implies A£u(t) E H. Thus, u(t) e D(A) and A£u(t) = Au(t). Consequently,
(23) is a solution of (24) and hence of (25).
The remaining assertions are obvious. 0
31.5. Application to Quasi-Linear Parabolic
Differential Equations
We \vant to solve the initial-boundary value problem
u, + Lu = 0 on G x JOt 00 [,
D"u(.,t) = 0 on oG x ]0, a:; [ for all p: IPI  m - 1, (26)
u(x t 0) = uo(x) on G,
with
Lu(x, t) = L (- I   ACI(.' Drl(x, t»
1«1 s "'
in an appropriate generalized sense. To this end, we will apply Proposition
31.13. We make the following assumptions:
(HI) G is a bounded region in R N , N  1. Let m  1.
We set H = L 2 (G) and V = Wplll(G) with 2 < p < OCt
(H2) The differential operator L satisfies the assumptions (H 1 )-(H4) of
Proposition 26.12 (Carathcodory condition, growth condition, mono-
tonicity and coerciveness condition).
We set
a(u, v) = f. L Acr(x, Du(x»DGrv(x) dx.
G 1«1 S lit
As usual, integration by parts yields the generalized problem for (26):
d
di (u(t)lw)H + a(r'(t), w) = 0 for all W E V. 0 < t < 00,
u(O) = Uo.
(27)

830 31. Maximal Accreti\'e Operators and Nonlinear Nonexpansivc Semigroups
By the proof of Proposition 26.12, there exists a monotone, continuous, and
coercive operator At:: V -. V. with
(AEu,v)v=a(u,v) for all u,veJt:
Furthermore, let D(A) == A E 1 (H) and Au = A£u on D(A). Motivated by
Proposition 31.13 we consider, instead of (27), the operator equation
u'(t) + Au(t) = 0, 0 < t < CO,
u(O) = Uo.
(28)
Proposition 31.14. Suppose that (H 1) and (H2) hold. Then:
(a) For each 110 E D(A), equation (28) laas a u,,;que solution in the sense of
Theorem 31.A.
(b) For each U o e D(A), equation (28) has the generalized solution u(t) = S(t)u o ,
",here {5(1)} is the nonexpansi,, sem;group ge'lerated b}' - A.
PROOF. This follows from Proposition 31.13.
o
Coro llary 31.15. If all the As are -functions, thell CO(G) s; D(A) and
D(A) = Lz(G).
The proof will be given in Problem 31.5.
31.6. A Look at Quasi-Linear Evolution Equations
We consider the quasi-linear evolution equation
11'(1) + A(u(t»u(t) = F(u(t» for all t E [0, T],
14(0) = 140'
where T > 0 is sufficiently small and the operator A(u): D(A(II»: X -. X is
linear and densely defined on the real B-space X. More precisely, we assume:
(H 1) Let X and Y be real reflexive B-spaces such that the embedding Y 5 X
is continuous and Y is dense in X. Furthermore, there exists a linear
isometric homeomorphism S: Y -. x.
(H2) Maximal accretivity. There is an open subset U of Y and a number fJ > 0
such that, for each u E U, the linear, densely defined operator
(29)
A(u) + pi: D(A(rl) S X -. X
is maximal accretive, and Y c D(A(u»).
(H3) Contractivity. For all u v e U and )' e Y:
II A (u»' - A(v))'lI x S const lIu - vllx 1I)7I1r,
II A (11))'11 x S canst II)' 11 y.

31.6. A Look al Quasi- Linear Evolution Equations
831
(H4) For each U E U, there is a linear continuous operator L(u): X --. X such
that supucv II L(Il) II < 00 and
SA(u)S-1 = A(u) + L(u).
(H5) The operator F: U -+ Y is bounded and, for allll, v e U,
111:(14) - f8(V)lI x  constllu - vllx.
(H6) For all u, v e U, w e X,
IIL(u)\" - L(v)\"'lI x  const Ii II - vllrI1\'lIx.
IIF(u) - F(v)lI r S const lIu - vII)'.
Theorem 31.8 (Kato (1976». If (HI)-{H5) ',old then, for eacl, Uo e U.there;s
aT> 0 such thallhe origillal problem (29) has a soilltion
u e C( [0, T], Y) '"' C 1 ([0, T], X).
Corollary 31.16 (Continuous Dependence of the Solution on the Initial Values)8
If, in addition, condition (H6) holds, then the map 1'0""'" u is continuous from U
into C([O, T]. y ,,,here T is locally constant on U.
An "elementary" proof of this important result can be found in Crandall
and Sougandis (1986). The idea is to prove the convergence of the difference
method
I't+l - Vt
t + A(V,)Vi+l = F(vj).
i = 0, 1, · · . , n,
(30)
Vo = "0'
where Vi denotes an approximation for u(it). For simplicity, let p = O. Since,
by hypothesis. the operator A(Vi) is maximal accretive. the inverse operator
(1 + t A(Vj»-1 exists on X and we obtain
V,+l = (1 + t A(Vi»-I(Vi + t F(Vi»).
The convergence proof for (30) is related to our proof of Theorem 57.A for
multivalued. maximal accretive evolution equations.
The original proof of Kato for Theorem 31.8 was based on the iteration
method
U+I (t) + A (u,,(t»u,,+ 1 (t) = F(un(t» on [0. T]
for n = 0, 1, .... In order to prove the convergence of (u,,) via the
Banach fixed-point theorem, one needs sharp results on linear semigroups.
Generalizations and important applications of Theorem 31.8 to nonlinear
partial differential equations in mathematical physics can be found in
Kato (1975), (1975a), (1976, L), (1985, L), (1986, S) and in Kato, Marsden, and
Hughes (1977) (second-order evolution equations). In this connection, quasi-
linear symmetric hyperbolic systems play an important role. This class of
problems will be studied in Chapter 83 by using another approach.

832
31. 4aximat Aocrctl\'C Operdtors and Nonlinear Noncxpansive Semigroups
31.7. A Look at Quasi-Linear Parabolic Systems
Regarded as Dynamical Systems
Many time-dependent problems in physics, chemistry, and biology can be
reduced to the following abstract quasi-linear first-order evolution equation:
U'(I) + A(t. U)II = F(I. u).
u(O) = 110'
O<IS1:
(31 )
where the operator "r.-. A(u, I)'" is linear. An important goal of modern
analysis is to develop a qllalitative tl.eor}' (or dynamical theory) for such
processes, parallel to the classical theory of dynamical systems with a finite
number of degrees of freedom (ordinary differential equations). The main
ingredients of such a theory should be the following:
(a) Existence and uniqueness of classical solutions.
(b) Existence of global smooth semiflows on appropriate state spaces in the
autonomous case (i.e., A and F are independent of time t).
(c) Blowing-up of solutions.
(d) Existence of global solutions for all times t > O.
(e) Stability of the time-evolution.
(f) Structural stability of the semiflow.
(g) Bifurcation.
F.or example, we are interested in the following questions with respect
to (e)-(g):
(i) Stability of equilibrium points.
(ii) Existence of limit cycles (stable periodic solutions).
(iii) Existence of attractors and repellers and their structure (e.g., their
Hausdorff dimension), and their stability under external influences.
(iv) Existence of stable, unstable, and center manifolds.
(v) Classification of structural stable semiflows.
(vi) Bifurcation of invariant sets under external influences (e.g., an equi-
librium point looses its stability and passes to a stable periodic solution
(Hopr bifurcation).
(vii) Classification of the roads to chaotic behavior (turbulence).
(viii) Classification of shock waves.
Simple typical examples for these phenomena have been considered in
Sections 3.7 and 3.8 in terms of ordinary differential equations.
Until today, a general theory is not availablc. The creation of such a theory
will be a huge program for the mathematics of the future. However, for general
processes of the reaction-diffusion type, we are able today to complete the
first important step towards a general theory. To this end, we consider fairly

31.7. A Look at Quasi-Linear Parabolic Systems Regarded as Dynamical Systems 833
general quasi-linear parabolic systems of the follo\\'ing form:
u, + ..(x, I, U)I' = J-(x, I, u) on G x ]0, 00[,
a(x, I, u)u = g(x, I, u) on oG x ]0, 00[, (32)
u(x,O) = uo(x) on G.
We want to show that, for given uo, there exists a unique maximal classical
solution, which forms a local semiflor in the autonomous case. A typical
application to reaction-diffusion processes will be considered in Example
31.17 below. In the following we sum over two equal indiccsj, k from 1 to N.
We set
u = (11 1 ,...,11.)
and x = (1"'.' N) with D i = alai as well as
d(x, I, u)u = - D)(aj.t(x, I, u)Dtu) + aJ(x, I, II)DJu
and either
&I(x, t, u)u = Q)t(x, t, u)1I j D,u,
(Neumann boundary condition) or
-2 = 1,
fM(x, t, u)r, = u,
2 = 0,
(Dirichlet boundary condition). As usual, n = (n 1 ,..., II,..) denotes the outer
unit normal to aGe
We assume:
(H 1) Let G be a bounded region in R N wi th oG e Coc and N > I.
(H2) All the coefficients are C 7J for x E G (resp. x E oG) and I > O. Explicitly,
this means that, for all j and k,
a, u j E C( G x R+ x (R"', R"'l),
f E (G x iii... x Rill, R'"), g E C.£J(oG x R+ x iii., R"'
where g(x, 1,0) = O. Note that ajt and a J are real (nJ x m)-matrices for
each fixed argument (x, r, u), whereas f and 9 are m-\'ectors.
(H3) Ellipticity of ..fII. For each (x, I, u) e G x R. x R- and D e R'" with
IDI = 1, all the eigenvalues of the (m x m)-matrix
Qjt(x, t, u)DjDIc
have positive real part
Recall that we sum over j, k = 1, .. ., N.
If a)t, aJ' f, and 9 are independent of time I, then we have the autonomous
case at hand.
As the state space we use
X = {u E Wpl(Gr: (1 - )u = 0 on oG},
where N < p < 00. Recall that u e W"I (Gr means that 14; E W p 1 (G) for all i.

834
31. Maximal Accretive Operators and Nonlinear Nonexpansi\'e Semi810uPS
Theorem 31.C (Amann (1988». Assume (HI)-(H3). Then:
(a) Existence and uniqueness. For each Uo E X, the original probleln (32) lIas
a un;qlle maxilnal classical solution 14 = u(t; 140). Let [0, t[ be Ihe maximal
half-ope" interval of existence, ,,,here t depends on Uo.
(b) Global existence. If ',"'e have tile a priori estimate
sup lIu(t; 1'0)11 x < 00
IEJ
for each bounded interval J = [0, T[ with T  t, tllen t = 00.
(c) Continuous dependence on the data. The solution U(l t > 0, depellds in
lhe topolog}' of X smooth I}' upon "0' a J 1c, ai' f, and g. For each II e X, lhe
functiolJ
ou(t; uO) h
tH
Olio
is equal 10 the solution of Ihe initial-boundary valr4e problem ,,,hich is
obtained by dWerentiating the original problem (32) formally \\lith respect
to Uo ill the directiolJ h.
(d) Local semiflow. In the alltonomous case, the map
(t, 1'0)'-+ II(t; 1'0)
represents a local semiflo"t on X.
(e) Bounded orbits. Suppose that there is a nunlber to E ]0, t,[ such that
sup lIu(t; uo)1I r < 00,
'oSt<:.
where Y = W:(G)'" for son,e fixed I > 1. TheIl l = 00, alld tIle orbit
{U(I;Uo): 0  I < co}
is relatively compact alld hence bounded in tIle state space X.
Set 11(1; rlo) = S(t)uo. Recall that the map in (d) is called a local semiflo".. iff
S(O) = I and
S(t + s)u o = 5(1)5(s)uo
for all Uo E X and for all t, s  0 for which these expressions exist. In addition,
we obtain that the map 1101-+ u(t; "0) in (d) is  on X for 0 S t < 1.,(Uo).
In very rough terms, the proof of this deep result proceeds along the lines
of the proof for Theorem 19.1, i.c., the abstract evolution equation (31) is
reduced to an integral equation via semigroup theory. In this connection, it
is very important, that the operator - A(u, t) generates an analytic semi-
group for each fixed argument (II, t). This follows from (H3) and reflects the
parabolicity of the problem. The detailed proof of Theorem 31.C is complex.
It is based on sophisticated results for linear elliptic and parabolic differential
equations, the theory of analytic semigroups and time-dependent first-order
evolution equations, interpolation theory, and the regularity theory for quasi-
linear parabolic equations. See Amann (1988), (1988a».

31.7. A Look at Quasi-Linear Parabolic Systems Regarded as Dynamical Systems 835
EXAt.tPI.E 31.17 (Chemotaxis Problems for Two Components). Let u = (u J , U2)
and x E R J . The results of Theorem 31.C can be applied to the following
semilinear parabolic system:
", - aAu = f(x, u) on G x ]0, 00[,
au
- a on = g(x, u) on cG x ]0, 00[,
(33)
u(X,O) = uo(x) on G,
\vhere
a = ( (XI fJa.2 ) .
o a2
Such equations describe reaction-diffusion processes in physics, chemistry,
biology, and population dynamics. The positive numbers a 1 and 2 are the
so-called diffusion coefficients, and the real number (J describes the drift of the
quantity U 1 caused by the gradient ofu2'
In order to obtain an interpretation in terms of chemistry let M i be the
mass of the itb substance, and let
Ut = JW,/(J\11 + M 2 )
be the concentration of the ith substance. The so-called current density vector
of diffusion for the ith substance is given by
J .. = - a.. g rad u..
I , .
An equation of the form
ou, d .. h
ot + IV), = 
means
d d r u,(x. t) dx = - f jjn dO + r hi dx, (34)
t J H J "" J "
for each reasonable subregion H of G. This follows after integration by parts.
Relation (34) describes the change of the mass of the ith substance in the region
H by diffusion via j; and by a source h., which corresponds to chemical
reactions. A more detailed discussion can be found in Section 69.1.
The original equation (33) can now be written as
aUt d .. r P d . ·
at + IV}1 =J1 - IVJz,

Ci" 2 d .. f G ]0 [
at + IV J2 = ;z on x ,CO t
with the boundary condition
j 1 n = 9 1 - pj 2 n,
j2 n =; (J2 on oG x ]0, 00[.

836
31. Maximal Accretive Operators and Nonlinear Nonexpansive Semigroups
and the initial condition
Ui(X,O) = UOi(X) on G, i = 1, 2.
The boundary condition describes diffusion through the boundary aGe
PROBLEt-'S
31.1. Mdx;mdl accretive operators. Let A: D(/I)  H ... H be a monotone operator
"'ith R(I + lA) = II for fixed i. > 0, where H is a real H-space. Show that A is
maximal accretive.
Solution: By Proposition 31.4. the operator A is accretive. Hence the operator
R). = (I + i.A) -1 is nonexpansive and it is sufficient to show that R(I .t- pA) ;;I H
for all Jl > O. The equation
u + pAu = "',
ue H.
is equivalent to
U  L...u,
ue H,
(35)
with Lwu  R,1((1 - p-l l )U + p-I AW For p > )./2, II - p-').I < 1 and
IILw u - L",vll S; II - p-l i.lllu - vII for all u, ve H.
By the Banach fixed-point theorem (Theorem I.A equation (35) has a unique
solution, i.e., R(I + pA) = H for all IJ > )./2. An n-fold repetition of this
argument yields
R(I + pA) = H
for all p > )./2" and all n.
31.2. Pr()(r of LemnuJ 31.8.
Solution: Let X = L 2 (O, T; H). The operator A is monotone. In fac for all
u, ( e D(A) the monotonicity of A implies
(All - AI7II1 - v)x - f: (AII(I) - AV(I)III(I) - vel)) dr  o.
We show that R(l + A) = X. Let '" eX. We set
U(I) := (I + A) - J ,,'(t),
Uo = (I + A)-1 (0).
Since A is maximal accretive, the operator (I + A)-I is nonexpansive, i.e.,
lIu(l) - uol1 2  11"'(1)11 2 . Integration over [0. T] yields u - Uo E X, i.e., U e X.
By Proposition 31.5, the operator If is maximal accretive and maximal
monotone.
31.3. l\ronexpansit'e operators and monotone operators. Let C: D(C)  H .... H be
a nonexpansivc operator. where H is a real H-space. Show that I - C is
monotone.
Solution: For all u, ve D(C),
«u - Cu) - (v - Cv)lu - v) = (u - vlu - v) - (Cu - Cvlu - v)
 lIu - v! 2 - IICu - Cvllilu - vii  o.
31.4. Proof of Corollary 31.1.

Problems
837
Solution:
(I) Let u o , l'O E D(A) and I, S > o. We show that
S(O)uo = 14 0'
S(t + s)uo = S(t}S(s)u o ,
IIS(t)uo - S(t)toll < lIuo - ('011,
1'-' S(t )u o is continuous on [0, oc [.
(36)
(37)
(38)
(39)
Let u(t) = S(t)uo. Then, by definition, u(.) is the unique solution of the
equation
u'(t) + Au(t) = 0,
o < I < 00, u(O) = Uo.
For fixed s  0 we set (1(1) = u(t + s). Then, v is a solution of the equation
("(1) + At(I) = 0,
o < t < 00, ('(0) = u(s).
Hence ('(1) = S(t)u(s). This is (37). Inequality (38) follows from (8), and (39)
follows from the continuity of u = u(t).
(II) Since 5(1): D(A )  D (A } is no nexpansive for allt  0, there exists a unique
extension 5(t): D(A)  D(A) such that (36)-(39) remain true. In fact, let
u E D(A) and let (u,,) be a sequence in D(A) with 14"  U as n  x,. Then
(u,,) is a Cauchy sequence. By (38), the sequence (S(I)U,,) is also Cauchy.
We set
S(t)u = lim S(I)U".
"-CX'I
Inequality (38) shows the independence of this limiting value of the
chosen sequence (u,,) and the uniformity of the limit process for allt  O.
Therefrom follow (36) through (39) also for the extended operators.
(III) By Step 9 in Section 31.3,
I . S(h)uo - Uo
1m h = - Au o
"-+0
for all Uo E D(A).
Let - B the generator of {S(t)} on D(A) . Then A c: B. By Problem 31.3,
(u - S(t)u - (L' - S(t)v)lu - L')  0 for all 14, t' E D(A) .
Differentiation at t = 0 yields (Bu - Bl'lu - v)  O. Hence B is monotone.
Since A is maximal monotone, B = A.
31.5. Proof of Corollary 31. t 5.
Solution: If all A are ca- -functions, then for 14 e CO(G), after integration by
parts, the inequality
la(u. vII = L [,Lu dx < const II ['II H
holds for all t' E Jt'. Therefore, there exists a '" E H with
a(u, l') = ("'ll)H
for all (' E V
Hence
a (14, l') = < w, v> v
for all v E Jt:

838
31. Maximal A(Xreti,'e Operators and Nonlinear Nonexpansivc Semigroups
On the other hand, a(u, v) rID (Alu. v)v for all v e  Hence A£u E II, i.e.,
u E D(A).
31.6. The generic propert)' of existence of solutions for differential equations on
B-spaces. Let X and Y be B-spaces over K = R, C. Let
I:: G  X .... Y
be continuous on the nonempty open subset G of X. Then there exists a
sequence (Fa) of locally Lipschitz continuous operators
F,,: G s; X -+ y
such that sup.tt G II Fu - F"u II -+ 0 as n -+ 00.
Hint: Use a partition of unity and set Fa u = L tVJ(u} FU Jt where "'J is an
appropriate real function. cr. Lasota and Yorke (1973).
DiS(.-uss;on. This result has the following important consequence. Along with
the original initial value problem
u'(I) = FU(I),
u(t o ) = U 01
we consider the approximate problem
U'(I) = fu(t).
I e V(t o ),
(40)
t e U(to
(41)
,,(t o ) = Uo.
Since F" is locally Lipschitz continuous. the approximate problem (41) has
locally a unique solution by Theorem 3.A. By Problem 31.6, the approximation
of f. by F. is arbitrarily close. Roughly speaking, this means the following.
Genericall}', tIlt initial value problem (40) has al\\Oa)'s a solut;on.
31.7.- l\'onexpansive semigroups on B-spaces mad initial t'alue problems. We consider
the initial value problem
U'(I) + AU(I) == 0, t  0,
u(O) = Uo.
(42)
We assume that the operator A: D(A) s; X  X is accretive on the IJ.space X
over K = (R. C, and that there is a i.o > 0 such that
D(A)  R(l + ,iA)
for all 0 < ;. < Ao.
For example, this condition is satisfied if A is maximal accretive. Then:
(a) The operator A generates a nonexpansive semigroup {5(t)} on D(A) by
means of
S(t)u = lim ( 1 + .!.A ) -"U
11-(6) n
for all t  0,
(43)
and all u e D(A). This s emig roup can be uniquely extended to a non-
expansive semigroup on D(A).
(b) If X is reflexive. then. for each Uo e D(A the initial value problem (42) has
the unique solution u(t) == 5(I)uo, where u'(t) exists for almost aUt e R..

References to the Literature
839
This fundamental result underlines the importance of maximal accretive
operators for the thcol}' of noncxpansivc scmigroups on B-spaces. A more
general result for multivalued maximal accretive operators will be considered in
Chapter 57. If X = LQ, then formula (43) means that S(t) := e-,A.
Hint: Ad(a), Cf. Crandall and Liggett (1971).
Ad(b). Cf. Brezjs and pazy (1970).
More recent results can be found in Crandall (1986, S) and Benilan, Crandall,
and Pazy (1989. M). cr. also Pavel (1987, M).
References to the Literature
Classical papers: Komura (1967), Crandall and Pal)' (1969). Crandall and Evans (1975).
Introduction to the theory of linear semigroups: pazy (1983. M).
Linear semigroups. interpolation theory" and approximation theory: Butzer and
Berens (1967. M).
Linear semigroups of positive operators: Nagel (1986, L).
Survey of the theory of nonlinear nonexpansive semigroups: Crandall (1986).
Nonlinear semigroups: Brezis (1973, L), Barbu (1976. M), Deimling (1985. M).
Clement (1987, M). Pavel (1987, M), Benilan, Crandall, and Pazy (1989, M).
Quasi-linear evolution equations: Kato (1975), (1975a), (1976). (1985. L), (1986,S).
Crandall and Sougandis (1986), Amann (1988), (1988a) (dynamic theory).
Quasi-linear e\'olution equations of hyperbolic type and mathematical physics:
Kato( 1975), Hughes, Kato, and Marsden (1977), Marsden and Hughes (1983" M),
Majda (1984, L), Christodoulou and Klainennan (1990, M
Quasi-linear evolution equations of parabolic type and reaction-diffusion processes:
Henry (1981, L), Amann (1984), (1985), (1986), (1986a-c), (1988), (1988a-c).
E\'olution equations, dimension of attractors, and Ljapuno\' exponents: Babin and
Viik (1983), (1983a. S (1986, S Temam (1986, S (1988, M Ladyunskaja (1987, S),
Iiale (1988, M).

CHAPTER 32
Maximal Monotone Mappings
Each symmetric operator can be extended to a maximal symmetric operator.
To a given maximal symmetric operator, there belongs none or exactly one
spectral family (i.e., the operator is not self-adjoint or it is self-adjoint).
John von Neumann (1932)
(Mathematical Foundations of Quantum Mechanics)
Let X be a Hilben space, with real or complex scalars, and with inner product
(ulv). For D  X. \\'C call (a not necessarily linear) operator A: D... X a mono-
tone operator provided that, for all  v e D,
(C) Re(Au - Avlrl - t:)  O.
We prove several properties of such operators, the most important of which arc
that the "integral equation of the second kind"
Au + u = b,
under a fe\\' additional hypotheses. always has a solution u for given b, and that
the solution depends continuously on b.
It is the author's feeling that some problems of mathematical physics could
be better reformulated in terms of the "graphs" of the operators-i.e., in terms
of a subset of the product space X xX....
The term "monotone" is preferred here because the theorems of this paper
resemble theorems on monotone nondecreasing real functions of a real variable.
George Minty (1962)
The reader should not be surprised at the appearance of multivalucd operators
in the theory of maximal monotone operators. They play an important role for
the following two reasons:
(i) A coherent theory of nonlinear nonexpansi'e semigroups necessarily de-
mands multivalued operators.
(ii) Certain classes of boundary value problems, particularly variational in-
equalities, are related to nondifTerentiable convex Cunctionals and these
equations can be described very conveniently by means of mullivalued
operators.
Haim Brczis (1973)
840

l\faximal Monotone Mappings
e

...
o
C)
.I::
eJ
>.
....
o
tAD
U
--

u .-.
.{I) 

.; 
=
<,n
...

><

>
C
8
'-'-''-e
 0
0\ C
... 0
--- --
I' ..
N 
0\ ::;
Q.

e

o
oU
.c
...
.c
u

C

=
!
c:
.s:: ....
 -
:c
...
c

.-
""0
cu
"-
00
&J
:s
CI)
'-
o
E
,-.
ON
U ....
.l::C\
-- 
eJ "'-'
c ...
.- u
8.
. =
." 0
u ...
a.(=

'-
o c
 -0.
 
 e
.  
c C
::I 0
o --
.c g
 
..J
.
9-

'-
o
>-
.. 
:g i
CO\
0_
-- "'-'
o
c :;
0-
E:!!
- cu

E U
.- 0
)(CI::
="-

.-.
N
:s
c. .... N
 "'-'
- s 
 II
eJ 
:.:: ::s
--
='
Q

i
0\
(J2-
"'-'
.- ..
... . 0
J2ca
.!! ;'-0
 c.. u C -.
.- C 
tJ._
C ..
.t: -; 
Q..c c
o C
U ._ ::I
U __ ..
c .D
u 'C 
;;Q
';( > '-'
UJ
-
'"
.5 
"c
'0 e ""s. Ci
0..00
C'- \O
.2 0 E 0\
;::.
N -- C ...
'C. 0 
"'eJ1'
U H 0 
f! . g 
E
U
..  
u c oU 
 .- > :s
.- Q. '(3
Q. ... g
8 e tlS 8 .:
u u ..
u >-c
- c - 0
a  .
:c c  oU
..... 0 .c --.
oe 04i
- >-c tII
.  e .::: 0 C
> > .. --
.- ._ .- 0 Q.
.. a< .. C c..
u  u 0 eIS
. Ii: - e E
= ='
 (I.)
-
tIS
e
.- en
>< CO
'" c
E -a.
'- c..
o 
= E
o
--
..
.. .
tIS
:;
cc
u
CI::
-
'"
e
.-
.c
'" 
Ec 
'- .- -a 0
o Q.CI'
C Q.CUO\
0 "'.-.-
e N --
.= \D..
 uO\CtS
S 'C c :, =5
-'u 0 >-....
o ..o--
C U c c:
o f 0-- g
e eeCI:
...
I U
g -0
o - 
e CtS e
o .5 e, <"
-0 3< Cl)N
  CCI
s. -s. e
C.8 Q.
o .. '" 0
S :s e u
... .I::
 .. U f-
o 8. 6 I
u...-.
.1::_000
--C
ccoO\
.- 0 e 
.. -

1.1"-"
c
o
..0\
o
C ...
ou.-.
e-o-.
c
o eo-.
ee,=
 
o c.:
 .
-- c..
c-g
-; e 
:E
-
.-.
o
I'
0\

-
...
E.!!
u-
....!!
o 
u
-= g
e
:s'-'
f.I)
<n
 .
o  .-
.= c g c;
'" 0 .- ='
::I .- .. a-
.... a- c; '" 
.".. U = g..:
ca"u-
.-  
u ... c c
.. 0
V) 0 ._ 0
..... ..'-
u  :s --
e ... '"
8. (5 'C
E > ="
 0 'U >
:c
841
....
N
tw\

:s
CO


842
---.
...0\
00\
co 00
u-"
 --
'" e
 f
. 2
.- .c
 ...
=
32. Maximal Monotone Mappings
.
-g F='
-o-oM
c_cO\
=' c. ccs ....
o .- --
.cu.cCl)
= u ='
e'e ." 
. ... c.;-a
  = .-
.-  
= .... 
::> 0 CI)
.-.
---.
cn\O
._ I"
. ;
e = )(
='--:s
CI)  '"
c...oL.
. 0 ; X

C D. C
c.s 0 '"

C e
(tJ =
:.;' E
E  .S
 " ....
I e 'g / e .!!
c - -c.
. C) 4.» 0 '" 0'-. f! · G
   c
.8   5 .
LIJ ...  0....
.c
u 
CCS 0\
C N
e2:
I  r:::
COM
.c",
..c::...
:C"
c.
c.s
e

...
.-
(;
='
Q
E.-.
M
0'"
O'.
.c....
.. 
eJ ....
= U
.- 
&.=
. 0
-gas
all( ....
.- 0
Lz..
c.f)

'- C
 . is.
o -; D.---.
.= e e 0
. 0= g 2:
UeO--
 .. (I)
. U ... O'N
f!C'f
"'cO 
.c .- E CD M
U - "'oIIP M

='
co
.-
tL.
= -0 0 .-01
o 1. .8 c 
SO"'OCM
U C =' e .- 
"'01:: Co.
ge8.<i5
 -=OeE
 .... ._ 0
C =' 8 »< u u
 g e 2 
o u
· ... C
gB-S.-
 a g °i 
'- .: 0 I Q
o D.E CI) JO
c c._ colt>
. .2 e e .: UI
 ._ a..-.
c u >< c.'"",
s--
..eEQ

E .-.
 <:>
... V'\
o 
IC)
... .- 
...O\ E
= C!S.... e
.- .t:J _
&. ; ....
· 0 en 
-g = u .c
)(.... C ...
.- 0  0
Lz.. N

32.1. Basic Ideas
843
The logical structure of this chapter is represented in Figures 32.1 and 322.
The ke}' to our approach is the main theorem on pseudomonotone perturba-
tions of maximal monotone mappings due to Browder (1968) (Theorem 32.A
in Section 32.4). This theorem will be proved via the Galerkin method.
Throughout this chapter, as an important auxiliary tool, we shall use the
method of regularization based on the duality map J. Here, the idea is to
replace the original equation
Au = b
,
" e X,
\vith
Au + £./" = b,
UE X,
and to consider the limit t.... + O. Figures 32. t and 32.2 show clearly that the
theory of maximal monotone operators is based on a number of fundamental
principles of Cunctional analysis. Typical applications of this theory concern
operator equations, evolution equations of first and second order, Hammer-
stein equations, and variational inequalities.
32.1. Basic Ideas
The notion of a ,naximal monotone operator is the ,nost importallt cOllcepl in
the theory of monotone operators. Each monotone operator possesses a
maximal monotone extension. The first basic result on maximal monotone
operators was proved by Minty (1962). This paper marks the beginning of
the modem theory of monotone operators. Minty proved that a monotone
operator
A: D(A)  X -+ X
on the real H-space X is maximal monotone iff
R(A + 1) = X.
In particular, each continuous monotone operator A: X -. X on the real
H-spacc X is maximal monotone, and A + I: X -. X is a homeomorphism,
i.e.. the equation
(1)
Au + u = b,
UE X.
(2)
has a unique solution U for each given b eX, and II depends continuously
on b.
32.1a. Typical Existence Theorems
The two main existence theorems of this chapter are due to Browder (1968),
(1968a), namely:
(i) the main theorem on pseudomonotone perturbations of maximal mono-
tone operators (Theorem 32.A); and

844
32. Maximal Monotone Mappings
(ii) the surjectivity theorem for maximal monotone operators (Theorem 32.G).
In the special case of single-valued operators on H-spaces, we obtain from
(i) and (ii) the following two results:
(i*) Let A: D(A) e X.... X be maximal monotone on the real H-space X,
and let B: X .... X be pseudomonotone, demicontinuous, and bounded.
Moreover, let B be coercive with respect to A, i.e., there is a U o E D(A) such
that
I . (But u - u o )
1m = +00.
till" - 7) " u II
Then, for each given b E X, the equation
Au + Bu = b,
U E X,
(3)
has a solution.
If we set B = /, then we obtain equation (2). Consequently, theorem
(i) generalizes the theorem of Minty mentioned above.
(ii*) Let A: D(A) e X.... X be maximal monotone. Then A is surjective iff
A-I: X .... 2 x is locally bounded, i.e., for each u EX, there is a neighbor-
hood U such that A -1 (U) is bounded.
Note that the surjectivity of A means that, for each b E X, the equation
Au = b
,
U EX,
(4)
has a solution.
In particular, if A: D(A) e X.... X is maximal monotone and
"Au" -. 00
as II u II .... 00,
then A is surjective.
This generalizes the following simple result. The monotone continuous
function A: R ..... R is surjective iff I Aul .... 00 as lul -.. 00 (Fig. 32.3).
For a linear operator A: D(A) e X.... X on a real H-space X, the following
two statements are equivalent:
(a) A is maximal monotone.
(b) A and A. are monotone, D(A) is dense in X, and A is graph closed.
- 
f--igure 32.3

32.1 BasIc Ideas
845
From Proposition 31.12 we obtain the following special case:
7.he 1i,lear sy,nmetr;c monotone operator A: D(A) c X -+ X on the real H-
space X is nlaxinlal monotone if! ;t is self-adjoint.
Thus, the theory of maximal monotone operators generalizes the classical
theory of self-adjoint operators due to John von Neumann. For example, this
theory can be found in v. Neumann (1932, M) and in Riesz and Nagy (1956, M).
I n Chapter 19 we have shown that linear self-adjoint operators allow
important applications to variational problems and to differential equations
of elliptic, parabolic, and hyperbolic type.
As we shall show in this chapter, the same is true for nonlinear maximal
monotone Dperators.
32.1 b. Typical Maximal Monotone Mappings
Let X be a real reflexive B-space. The following examples show that many
important operators are maximal monotone:
(a) Protot ype. If A: .¥  X. is monotone and hemicontinuous, then A and
A -1: X. --+ 2,t are maximal monotone.
(b) Gradients. If the CI-functional f: X --+ R is convex, then the derivative
I': X --+ X. is maximal monotone.
(c) Subgradients. If .f: X -+ ] -.'XJ, :.] is convex and lower semicontinuous
with f  + 00, then the subgradient (Y: X -+ 2 x . is maximal monotone
(Theorem of Rockafellar ( 1966)).
In particular, iff: X -+ R is G-differentiable, then cJ"(u) = {.l'(u)} for all
u EX, i.e., the subgradient eneralizes the G-derivative.
(d) The duality map J: X -+ 2 x defined through
J u = i( 2 -I "u 11 2 ) for all u EX,
is maximal monotone.
In particular, if X. is strictly convex (e.g., X is an H-spacc), then J = I'
where f(u) = 2- l lIull 2 ,
(e) Tinle derivatit'es. We consider functions of the form u: [0, T] -.. V, where
o < T < T.J. Let
X = Lp(O, T: '),
1 < p < 0Ci,
where uv c H c V." is an evolution triple. We define the operator
Lu = 14"
where either
D(L) = {u E W p l (0, 7.: V, H): u(O) = O}
or
D(L) = {u E Wpl(O, T; V, H): u(O) = u(T) = O}.

846
32. Maximal Monotone Mappings
Then the operator
L: D(L) c X -+ X.
is maximal monotone. Recall that X. ...:. L,(O, T; V.), where p -I + q-l = I.
(f) Regularization and generators of semigroups. Let X and X. be strictly
convex, and let A: X -+ 2 x . be monotone. Then, A is maximal monotone iff
R(A + AJ) = X
for all A > 0
(5)
(Theorem of Rockafellar (1970)). This result is the basis of an important
regularization technique to be discussed below.
In the special case of an H-space X, we may identify X. = X. Then
J = I (the identity on X). Thus, (5) generalizes the Theorem of Minty
mentioned in (I) above.
By Theorem 31.A, each maximal monotone operator A: D(A) c X -+ X
on a real H-space X has the property that - A generates a nonexpansive
semigroup {S(t)} on D( A). Letting
u(t) = S(t)uo,
we obtain generalized solutions of the initial value problem
u' + Au = 0,
u(O) = uo.
(6)
In order to obtain a complete characterization of nonexpansive
(nonlinear) semigroups on H-spaces, one needs multivalued maximal
monotone operators. The precise formulation can be found in Section
32.24.
(g) Sum rule. Let A, B: X -+ 2 x . be maximal monotone and suppose that
D(A) (\ int D(B) :1= 0.
Then the mapping A + B: X -+ 2 x . is maximal monotone (Theorem of
Rockafellar (1970)).
32.1c. Typical Applications
In this chapter we study the following applications:
Hammerstein operator equations and Hammerstein integral equations;
evolution equations of first and second order (initial value problems as
well as periodic problems);
variational inequalities of elliptic and parabolic type.
Applications of variational inequalities to elasticity, plasticity, hydrodynamics,
gas dynamics, optimal control, etc., will be considered in Parts III through V.
Let us explain some basic ideas. The details will be studied below in this
chapter. The main theorem on perturbed maximal monotone mappings of
Browder in Section 32.4 concerns the solvability of the fundamental equation
bEAu + Bu, u E C, (7)

32.1 Basic Ideas
847
where A: C -+ 2 x .is maximal monotone and B: C -+ 2 x . is pseudomonotone.
Moreover, C is a nonempty closed convex subset of X. The proof of this
fundamental result is based on the existence principle for inequalities
in Section 2.11 (the extension theorem of Debrunner and Flor (1964) for
monotone sets). This principle follows from the Brouwer fixed-point theorem.
In the following, we want to show that completely different problems can be
reduced to equation (7).
Hammerstein Equations
To solve the equation
u + KFu = h,
U E X,
(8)
we use the multivalued inverse mapping K- 1 . This way we obtain the
equivalent problem
OeK-1(u-b)+Fu,
which is of the form (7).
U E X,
(8.)
First-Order Evolution Equations
The initial value problem
u' + Bu = b,
can be written in the form
u(O) = 0,
(9)
Au + Bu = h, u E X, (9*)
where Au = u' with D(A) = {u E Wpl(O, T; Jt:H): u(O) = O} and X = Lp(O, T; V).
Similarly, the following evolution equation with periodicity condition
u' + Bu = b,
u(O) = u(T) = 0,
(10)
leads to the problem
Au + Bu = b,
U E X,
( 10*)
where Au = u' and D(A) = {u E Wpl(O, T; V, H): u(O) = u(T) = O}.
Reduction of Second-Order Evolution Equations
to First-Order Evolution Equations
We consider the initial value problem
utI + Nu' + Lu = b,
u(O) = u'(O) = O.
( 11 )

848
32. Maximal Monotone Mappings
Letting v = u', we obtain the first equivalent problem
Vi + Nv + L(f V(S)dS) = b, v(O) = O. (II.)
Again letting v = u', we obtain from (") the second equil,ulent problem
u' - v = 0,
v' + Nv + Lu = b,
(11..)
u(O) = 0,
v(O) = o.
Letting \\' = (u, v), problem (II..) can be written in the form
",' + Bw = (0, b),
w(O) = O.
Note that (".) and (II..) represent first-order evolution equations. Equation
(II.) (resp. (II..) will be used in Chapters 32 and 33 (resp. Chapter 56).
Free Minimum Problems
The minimum problem
f(u) = min!,
U E X,
( 12)
for the functional f: X --. IR is equivalent to the Euler equation
o E iJf(u),
This problem is of the form (7) with A = af.
Constrained Minimum Problems
U E x.
( '2.)
Let C be a nonempty closed convex subset of the real reflexive B-space X, and
let f: X -+ R be convex and lower semicontinuous. Define
x(u) = { o
+0Ci
if u E C,
if u f Co
Then the minimum problem
JO(u) = min!,
is equivalent to the Euler equation
o E of(u) + (lX(u), u E X. (13.)
By the Theorem of Rockafellar (c) above, the mappings of, ax: x -+ 2 x . are
maximal monotone. By the sum rule (g) above. the mapping A = of + ox is
maximal monotone if int C ¥- 0. Hence problem (13.) is of the form (7).
If .f: X --+ R is G-differentiable, then (13.) is equivalent to the variational
U E C,
( 13)

32.1 Basic Ideas
849
inequality
<f'(u), l' - u) > 0
for all l' E C.
( 13..)
Here "'e seek U E C. Problems of the form (13) will be studied in detail in
Chapter 47 in the context of convex analysis.
Elliptic Variational Inequalities
Instead of (13..), we consider the more general problem
<Bu - b, t' - u) > 0,
for all (J E C,
( 14)
where we seek U E C. This is equivalent to the equation
b E (1X(u) + Bu,
U E C,
(14.)
which is again of the form (7) with A = x.. In the special case C = X, the
variational inequality (14) for the operator B: X --+ X. is equivalent to the
operator equation
Bu = b,
U EX.
Evolution Variational Inequalities
We set Lu = u', where D(L) is given as in (e) above and X = L 2 (O, T; V). If we
replace the operator B in (14) with Lu + Bu, then we obtain a so-called
variational inequality:
< Lu + Bu - b. v - u) > 0
for all [' E C,
( 15)
where we seek u E C. This problem is equivalent to the equation
b E CX(u) + Lu + Bu,
U E C.
(15.)
The operator L: D(L} c X -+ X. is maximal monotone. By the sum rule (g)
above, the mapping A = i'IX + L is maximal monotone if int C :i: 0.
M ore General Varia tional I neq uali ties
Let qJ: X --+ ] - X'. 00] be a convex lower semicontinuous function with cp 
+. We consider the variational inequality
<h - Bu. l' - u) + cp(u) < qJ(l'}
for all v EX,
( 16)
where we seek u E C. This problem is equivalent to the equation
b E ocp(u) + Bu,
U E C.
( 16.)
In the special case cp = X' problem (16) is equivalent to (14).

850
32. Maximal Monotone Mappings
Method of Regularization
Along with the original problem
beAu + Bu,
we study the regularized problems
b e AI'r: + £Ju + Bu" u, e X (17*)
for small ,; > o. Let A: X -+ 2 x . be maximal monotone and let B: X -+ X* be
pseudomonotone. Suppose that X and X* are strictly convex. Then the
inverse operator (A + ,;J)-l: X. -. X is single-valued, and (17*) is equivalent
to the equation
ue X,
(J 7)
u& = (A + rJ}-I(b - Bu,J,
u, eX.
(17.*)
Suppose that, for each small £ > O equation (17*.) has a unique solution U t
(e.g., B = 0). In Section 32.18 we shall Connulate conditions which guarantee
that, as t  +0, the sequence (u,) converges to a solution u of the original
problem (17). Summarizing, we obtain:
The method of regularizatioPJ allo\\'s us to solve nonuniquel}' solvable equa-
tions by naeans of a family of uniquel}1 solvable equations.
32.2. Definition of Maximal Monotone Mappings
We first consider some basic notions for multivalued mappings.
Definition 32.1. Let
A: M.... 2 r
be a multit'alued mapping, i.e., A assigns to each point U E M a subset Au of 1':
(i) The set
D(A) = {u e M: Au  0}
is called the effective domain of A.
(ii) The set
R(A) = U Au
lieN
is called the range of A.
(iii) The set
G(A) = {(u,v)eM x Y:ueD(A),veAu}
is called the graph of A. Instead of (u, v) e G(A} we briefly write
(II, v) E A.

32.2. Definition or Maximal Monotone Mappings
851
The inverse mapping
A-I: Y-.2 Jf
is defined naturally enough by
A- 1 (v) = {u E M: ve Au}.
Obviously, we have D(A -I) = R(A) and
(u, v) E A iff (v, u) E A-I.
Let X and Y be linear spaces over t( and let M c X. For given maps
A, B: M ..... 2 r
and for fixed  {J e IK, we define the linear combination
«A + fJB: J\1 -+ 2 Y
through
( A fJB» { t%AU + pBu
IX + (u = 0
Obviously, D(cxA + PB) = D(A) n D(B).
In terms of set theory. the multivalued map A: M -. 2 Y is a subset of M x y:
In this sen the graph G(A) is identical to the subset A of M x t:
Each single-valued map
if u e D(A) n D(B),
otherwisc.
A: D(A) 5 .,." -+ y
can be identified with a multivalued map
A: M  2 Y
by setting
Au = { {Au} if U e D(A
o otherwise.
Then D(A) = D(A) and R(A) = R(A). In the following, single-valued maps
will always be regarded as multivalued maps. Instead of A we will brieny
write A.
The map B: M -. 2 Y is called an extension of A: M -+ 2 Y iff G(A) c G(8).
The following definition is basic.
Definition 32.2. We consider the multivalued map
A: M ..... 21'.,
where !vI is a subset of the real B-space X.
(a) A subset S of M x X. is called monotone iff
(18)
(U* - v., u - v)x  0
for all (u, u*), (v, v*) E S.

852
32. Maximal Monotone Mappings
(b) A subset S of M x X. is called max;nJal monotone iff it is monotone and
there is no proper monotone extension in M x X..
(c) The map A in (18) is called nJonotone iff the graph G(A) is a monotone set
. M 1.'..
I n x A , I.e.,
(u. - 1'.,14 - ") x  0 for all (u, u.), (v, v.) E A.
(d) The map A in (18) is called nJax;,nal monotone iff the graph G(A) is a
maximal monotone set in M x X., i.e., A is monotone and it follows from
(u. u.) E M x X. and
(u. - v., u - v)x > 0
that (u, ".) E A.
for all (v, v.) E A
An operator
A: D(A) c X  X. (19)
is to be understood as a multivalued map A: X -+ 2 x .. Thus, A in (19) is called
monotone iff
(Au - Av, u - v) x > 0
for all u, v E D(A).
(20)
Furthermore, A in (19) is called maximal monotone iff A is monotone and it
follows from (14, u.) E X x X. and
(u. - Av, u - v)x > 0
for all v E D(A)
(21 )
that u E D(A) and u. = Au.
If X is an H-space, then we identify X. with X in the sense of the Identifica-
tion Principle 21.18. Thus, we have to replace (', . ) x by the scalar product
( . I . ) on X . For example, a set S in M x X is called monotone iff
(u. - (>., u - v) > 0
for all (u, u.), (v, v.) E S.
The map
A: M  2 x
with M c X is called maximal monotone iff
(u. - v., u - v) > 0
for all (u, u.), (v, l'.) E A,
and it follows from (u, u.) E M x X and
(u. - v., u - ") > 0
for all (v, v.) E A,
that (u, u.) E A.
EXAMPI.E 32.3. Consider the H-space X = . A set S in X x X = 2 is
monotone iff
(u. - v.)(u - (1) > 0
for all (u, u.), (v, v.) E S,
.
I.e.,
(u, u.), (v, v.) E S
and
u<v
implies
u.  v..

32.2. Definition of Maximal Monotone tappings
853
L
s
(a) monotone set in R 2
(b) maximal monotone
set in R 2
(c) nonmonotonc: set in R 
Figure 324
The set S in Figure 32.4(a) is monotone in [R2, whereas S in Figure 32.4(c) is
not monotone in (R2. Furthermore, S in Figure 32.4(b) is a maximal monotone
set in R 2 , since there is no proper monotone extension of S in (R2.
EXA"fPLE 32.4. We consider the function
f: R -+ R
(22)
on the H-space X = IR. Then:
(a) If f is monotone increasing and continuous, then J'is maximal monotone
(Fig. 32.5(a). Indeed, it follows from (u. u*) E R 2 and
(u. - f(v)(u - v)  0 for all v e R,
that u. = f(u). Argue by contradiction.
.
.
f
f
-- - - -.
(a) maximal monO(one mapping
(b) monotone ntapping
(noc maximal monotone)
+
/
(c) maxintal monotone mapping
Figure 32.5

854
32. Maximal Monotone Mappings
(b) However, the monotone increasing discontinuous function f in Figure
32.5(b) is a monotone map, but not maximal monotone, since the graph
of f possesses a proper monotone extension in R 2 pictured in Figure
32.5(c).
(c) The map f: R --. 2 R pictured in Figure 32.5(c) is maximal monotone.
Proposition 32.5. The map A: X -+ 2 x . on the real rej7exive B-space X IS
maxilnal monotone iff the inverse map A-I: X. --. 2 x is maximal monotone.
Here, we identify X.. with X.
PROOF. This follows from
G (A - I ) = {( u., u) EX. X X: (u, u. ) E G (A) }
and from (u., u)x = (u, u. )x. for all (u, u.) E X X X.,
D
Proposition 32.6. If A: X -+ 2 x . is maximal monotone on the real B-space X,
then the set Au ;s convex and weakly. closed in X. for each u E X.
PROOF. Let u, uf E Au. Set u: = (1 - t)u + t ur, 0  t  I. For all (v, v.) E A,
(u: - v., u - v) = (I - t)(u - v., u - v) + t(uT - v., u - v)
> O.
Hence u: E Au.
Let (u:) be an M -S sequence in Au such that u:  u. in X*. It follows from
(u: - v., u - v)x > 0
for all (v, v.) E A,
that (u. - v., u - v)x > 0 for all (v, v*) E A. Hence u. E Au.
D
32.3. Typical Examples for Maximal
Monotone Mappings
32.3a. Single-Valued Monotone Operators
Proposition 32.7 (Prototype). Let A: X -+ X. be a monotone hemicontinuous
operator on the real rej7e.'(ive B-space X. Then A and A -I: X. -+ 2 x are maximal
monotone.
Here., we identify X.. with X.
PROOF. The maximal monotonicity of A follows from the monotonicity
trick (25.4), and the maximal monotonicity of A -I follows from Proposition
32.5. 0

32.3 TYPical Examples for Maximal Monolone Mappings
855
Proposition 32.8 (Minty (1962)). A monotone operator A: D(A) c X  X on the
real H-space X is maxinlal monotone iff R(A + I) = X.
PROOF. This is a special case of Theorem 32.A below. More precisely, if
R(A + I) = X, then A is maximal monotone by Proposition 31.5. Conversely,
if A is maximal monotone, then R(A + I) = X by Corollary 32.25. 0
Corollary 32.9. If the operator A: X -+ X is monotone and continuous on the
real H-space X. then A + I: X  X is a homeol1Jorphism.
PROOF. By Proposition 32.7, A is maximal monotone. Hence R(A + I) = X.
By Proposition 31.5, the operator (A + I)-I: X  X is nonexpansive. 0
32.3b. Time-Derivatives
We define
L 1 14=u',
D(L 1 ) = {u E W p 1 (O, T; V,H): u(O) = a},
D(L 2 ) = {u E W,l(O, T; V,H): u(O) = u(T)}.
(23)
(24)
L 2 u = u',
Proposition 32.10. Let "V c H c v. n be an evolution triple and let
X = Lp(O, T; V),
"'here I < P < XJ and 0 < T < 00. Then the two linear operators
L i : D(L i ) C X  X.,
i = 1, 2,
are maximal monotone.
Recall that X. = L,,(O. T; V*), where q-I + p-I = I.
PRC)()F. We make essential use of the integration by parts formula
!aT (u'(t), U(t)Vdt = rl(lIu(T)II - II u(O) II i,),
for all u E W p l (0, T; V, H).
(I) Obviously, L; is linear.
(II) L i is monotone. Indeed, it follows from (25) that
(Liu,u)x = 2- 1 (lIu(T)lIh - lIu(O)II)
(25)
(26)
for all u E D(L i ). Hence (Liu,u)x > 0 for all u E D(L i ).
(III) L i is maximal monotone. To pr.ove this, suppose that (v, w) E X x X* and
o < (w - Liu, " - u)x
for all U E D(L i ).
(27)
We have to show that l' E D(L i ) and w = Liv, i.e., ' = v'.

856
32. Maximal Monotone Mappings
To this end, choose
U = qJZ, where qJ e C:(O. T) and z e V.
Then u' = tp'z and II E D(L i ). By (26), (Liu,u) = O. From (27) it follows
that
o < (w,v)x - f: (lp'(t)v(t) + lp(t)"'(t), z).,dt, (28)
for all Z E V. In this connection. note that (a, b)v = (b, a)v for all a, b e V
by (23.17). From (28) we get
fOT lp'(t)v(t) + lp(t)w(t)dt = 0 for all lp e q'(O. T).
Hence
v' = ,,,.
and v E W p J (0, T;  H), since \V E X*.
It remains to show that v e D(L.). Using integration by parts, we
obtain from (27) that
o S (v' - II', V - u)x
= 2-- 1 (lIv(T) - u(T)n;, - IIv(O) - u(O)[I;,). (29)
Ad LI. Choose a sequence (a.) in V with Tall  v(T) in H as II -. 00. Set
II(t) = ta... Then II e D(L 1). By (29), v(O) = 11(0) = O. Hence v e D(L 1 ).
Ad L2,. From (29) we obtain
o s IIv(T)ni, - 'v(O)lIi + 2(II(O)Iv(0) - v(T»",
for all U E D(L2,). Note that 11(0) = u(T). In particular, we can choose u(t) = a
for arbitrary a E  Hence v(O) = veT), i.e., v e D(L 2 ). Note that V is dense
in H. 0
32.3c. Subgradients
Subgradients generalize the classical concept of a derivative. In this connec-
tion, the following formula
I(v)  feu) + (U., v - u)x
for all veX
(30)
is crucial.
Definition 32.11. Let f: X -. [ -00. 00] be a functional on the real B-space 'X.
The functional u. in X. is called a subgradient of f at the point II iff
feu) :/= + 00 and (30) holds.
The set of all subgradients of f at u is called the suhdifferential of(u) at II. If

32.3. T)'picaJ Examples for Maximal Monotone Mappings
857
/
/
/'
/
/
----H
;'
/
/
,;'
II
Figure 32.6
no subgradients exist, then we set of(l4) = 0. In particular, let of(u) = 0 if
f(u) = :too.
If Of(14) #: 0, then f(v) > -00 for all v E X, by (30). Subgradients will be
considered in detail in Part III.
EXA1tIPLE 32.12 (Real Functions). For a function f: R ..... R. the subditTerential
at u equals the set of all slopes u. E R of straight lines through the point
(u,f(u» which lie below the graph orf(the generalized tangents in Figure 32.6).
If the derivative f'(u) exists. then of(u) is single-valued, i.e.,
af(u) = {f'(u)}.
(31)
We now want to generalize relation (31) to B-spaces.
Proposition 32.13. Let f: X --. R be a functional 0'1 the real B-space X. Theil:
(a) If f is convex and if f possesses tlae G-derivative f'(u) at the point u, ll,en
(31) laold.".
(b) Conversel}', if of: X -. X. is single-valued and hemicontinuous, then f is
G-differentiable on X and (31) holds for all u e X.
PROOF. Ad(a). Set (j)(t) = f(u + th). Since tp: R -. IR is convex, q>(I) - cp(O) 
tp'(O). Hence
f(u + h) - f(u)  (/'(u), h) for all IJ e X,
i.e., f'(II) E C f{u).
Conversely, if u. e of(u), then
J(u + tll) - f(u)  (u., th) for all hEX, t > 0,
and hence (f'(u), h) > (u., h) for all heX. This implies u. = f'(u).
Ad(b). Let t > O. From
f(u + th) - feu)  (of(u'hrh),
f(u) - feu + th) > - (iJf(14 + III), th),

858
32. Maximal Monotone Mappings
we obtain
<of(u).h) S lim f(u + th) - f(u)
r+O t
S Jim f(u + lh) - f(u) S <of(u). h>.
,-'+0 t
for all hEX, i.e., f'(u) = o/(u).
o
Proposition 31.14 (Minimum Principle). Let f: X -+ ] -00, <X)] be a functional
on the real B-space X '\lith f ¥ + 00. Then u is a solution of the minil1wm
problem
f(u) = min!,
ue X,
(32)
iff
o E of(u).
(33)
PROOF. This follows immediately from (30).
o
Intuitively, the Euler equation (33) means that there exists a horizontal
hyperplane H through the minimal point (u,f(u» such that the graph of flies
above H (Fig. 32.6).
EXAMPLE 32.15 (Support Functionals of Convex Sets). Let C be a nonempty
closed convex set in the real B-space X with the indicator function X, i.e.,
{ o if ueC
X(u) = +00 if u e X-C.
By a support functional to C at the point u we understand a functional u. in
X. such that
(14*,11 - v) > 0
for all v e C.
(34)
Obviously, if u is an interior point of C, then (34) is equivalent to u. = o.
Generally, the equation
(u., u - v) = 0, veX,
describes a closed hyperplane H in X through the point u. Thus, relation (34)
means that the set C lies on one side of the hyperplane H (Fig. 32.7). According
to (30), we obtain:
a (u) = { set of all support functionals to C at u
X 0 if ueX-C.
In particular,
if U E C;
ox(U) = to}
Moreover, we have 0 e Ox(u) if u e C.
if u e int C.

32.3. Typical Examples lor Maximal Monotone Mappings
859
+ I
I
C 1 H
I .;'
I ",,"
"
"1 U
"",
.;" I
", 1
I
.
Figure 32.7
The mappings
.
aX: C --. 2 x
(35)
and
.
aX: x --. 2 x
(36)
are maximal monotone.
.
PROOF. Ad(3S). We show first that ox.: C  2 x is monotone. Let u. e OX(u)
and v. E CX(v), where 14, v e C. Then, for all w e C,
(u., " - ",)  0 and (v., v - ",)  O.
Hence (u. - I'., U - v) > O.
.
Next we show that aX: C .... 2 x is maximal monotone. Suppose that (II, u*) e
C x X. and
(u. - v..u - v)  0 for all (v, v.) e aX, (37)
i.e., v e C and v. e OX(v). Note that 0 e aX(v) for each v E C. Letting v. = 0 in
(37). we obtain
(u.," - v)  0 for all v E C, i.e., u. E OX(u).
Ad(36). This is a special case of Proposition 32.11 below. 0
.
Definition 32.16. The mapping A: X ..... 2 x on the real 8-space X is called cyclic
monotone iff the inequality
(1IT,111 - "2) + (U!,U2 - "J) + ... + (u:,u" - U,,+I)  0
holds for all (u it ur) e A, ; = I...., n, and all n E N, where we set U,,+1 = Ul.
Furthermore, A is called maximal cyclic monotone iff A is cyclic monotone
.
and there is no cyclic monotone mapping B: X ..... 2% such that G(A) c: G(B).
We make the following assumption:
(H) Let f: X .... ] -00. 00] be convex and lower semicontinuous on the real
a-space X and let f  +00.
Recall that f is called convex iff
1«1 - t)u + tv) S (1 - t)f(u) + tf(v)

860
32. Maximal Monotone Mappings
for all u, v EX, I E ]0, 1 [. Moreover, f is called lo,,'er sem;co",;nuous iff the set
{II e X: .((11) S r} is closed for all r e R.
For example, condition (H) is satisfied for each continuous convex func-
tional f: X -. R.
Proposition 32.17 (Rockafellar (1966». If (H) holds, thell tile subgradient
.
cf: X -+ 2 x is maxinJal nJOlJoto'le.
.
Corollary 32.18 (Rockafellar (1970b»). For a mappi'lg A: X -. 2-' 0'1 tlte real
B-space X, the follo'i'lg t,,o assert;olJs are equivalent:
(i) A = of and f satisfies (H).
(ii) A is maximal c)cl;c monotone.
PROOF. This foJlo\vs from Theorem 47.F, which we prove in Section 47.11 b)'
using the separation of convex sets. 0
Corollary 32.18 reflects the fact that not all maximal monotone operators
are subgradients.
32.3d. Duality Map
The duality map represents an important auxiliary tool in the theory of
maximal monotone operators.
Definition 32.19. Set tp(u) = 2- 1 11u1l 2 for all u E X, where X is a real B-space.
The duality map
.
J: X -+ 2 x
of X is defined to be J = acp.
EXAtPLE 32.20 (Prototype). Let X be a real H-space. Then the duality map
J: X -+ X. is single-valued and
(Ju, v) = (ulv)
for all u, v EX.
PROOF. Set t/J(l) = (u + th) = 2- 1 (11 + thlu + th). f e IR. Then 1/1'(0) = (ulh)
and hence (tp'(u),h) = (ullJ) for all heX. By Proposition 32.13(a), J = tp'.
o
Example 32.20 shows that, in the case of H-spaces, Definition 32.19 is
equivalent to our earlier definition given in Section 21.6.
.
Proposition 32.21. The duality nUlp J: X -+ 21' of a real B-space X is nul:(imal
monotone and cyclic monotone.

32.3. Typical Examples for Maximal Monotone Mappings 861
PROOF. This follows from Proposition 32.17 and Corollary 32.18. D
Proposition 32.22 (Nice Properties of the Duality Map). Let X be a real
reflexive B-space, and let the dflal space X. be strictly con(,x. Set tp(u) =
2- 1 11u1l 2 for all 14 e X. Then:
(a) The dI4alit}' 'nap
J:X-.X*
is ..ingle-valued. surjective, odd, demicontinflous, maxi"Jal nJonoto'le, bounded,
and coercive.
For all u e X, ,,'e have
Jfl = tp'(u),
(38)
",lIere cp' dellotes tile G-deriL'tllive. Tlnls, J is a potential operator ,,'itla
potential tp.
TIle norm U'--' lIull is G-difJerentiable on X - {O}. If \ve set "'(u) =
II u II, t I,ell
\I1'(u) = I:I
for all u E X - {O}.
JWoreover, for each 14 E X, there exists e.'(actl}' one .functional u. e X.
sucla that
(u*, u)x = lIu*lIlIuli
and
1114*11 = lIuli.
(39*)
Tile functional 14* is equal to J u. Thfls, for all u EX,
(Ju, u)x = 11"11 2 and IIJull = lIuli.
(39)
Moreot'er, J is positively 1J0"wgeneous, i.e..
J(AU) = )"Ju
for all A > 0, 14 eX.
(b) Let X be strictly cO'lvex. Then the operator J: X -. X* is stricti}' nJOlJotolJe
and bijective. The inverse operator
J- 1 :X*-.X
is equal to the dualit}' map of the dual space X* provided we identif}' X**
,,'ith X.
Furthermore, it follo,."s IronJ
(Ju. - Ju, un - u>x -+ 0 as n..... 00, (40)
that Un  II in X as n ..... 00. If, in addition, X is locally unifor,nly convex,
the" (40) in'plies 14" -+ 14 as IJ -+ X), i.e., J satisfies condition (S).
(c) Let X* be locally uniformly convex. The'l J: X ..... X. is continuous.
f.",IlJermore, the norm u......llull is F-difJerentiable on X - {O}.
(d) Let X and X* be locall)' uniform/}' conL'eX. Then the duality map J: X ..... X*
is Cl homeomorplJisnJ.
(e) Let X. be uniforml)' cOIJvex. The" J: X -+ X* is 14niformly continuous on
bOlllJded subsel. of x.

862
32. Maximal Monotone Mappings
The F-derivative of the norm ut-+ IIuli is uniformly continuous on bounded
subsets of X that lie outside some neighborhood of the point u = O.
Recall that, for each B-space Y, we have the following implications:
Y is uniformly convex -. Y is locally uniformly convex
1 !
Y is reflexive Y is strictly convex. (41)
!
Y. is reflexive
The corresponding definitions may be found in Section 21.7. In this connec-
tion, the following deep result of the geometry of B-spaces is important.
Proposition 32.23 (Kadec (1959) and Troyanski (1971)). In every reflexive
B-space X, an equivalent norm can be introduced so that X and X. are locally
uniformly c:onvex and thus also strictly convex with respect to the new norms on
X and X..
PROOF. Cf. Cioranescu (1974, M), p. 98.
D
Combining Propositions 32.22 and 32.23, we obtain the following result
which we will use frequently:
Corollary 32.24. Let X be a real reflexive B-space. Then we can introduce an
equivalent norm on X so that, with respect to the new norms on X and X., the
following holds true. The duality map
J: X --+ X.
is an odd homeomorphism. Moreover, J is strictly monotone, maximal monotone,
bounded, coercive, and J satisfies condition (5). For all u, v E X, the relations
(38) and (39) above hold. The inverse operator J- 1 : X. -+ X is the duality map
01 I lie dual space X..
Consequently, in considerations that are independent relative to passing to
an equivalent norm on X, one can always assume that the duality map J
possesses the propitious properties given in Corollary 32.24.
PROOF OF PROPOSITION 32.22(a).
.
(I) We define the map A: X --+ 2 x through
Au = {u. EX.: (u.,u)x = lIull 2 , lIu." = lIull}.
We first show that Au :F 0. Let Xo = span{u}. We set
f(tu) = III u 11 2 for all I E R.
Hence, I: X 0 --+ R is a linear functional with norm IIfll = II u II. By the

32.3. Typical Examples for t-taximal Monotone Mappings
863
Hahn-Banach theorem, we may extend f to a linear continuous func-
tional u.: X -+ IR with nu.1I = lIuli. Obviously, (u.,u) = f(u) = 1114"2.
Next we show that A is single-valued. To this end, let ur e Au, i =
I. 2, i.e.,
( II r , 14) = II U 11 2 = II u  11 2 .
i = 1, 2.
Hence
2Uufliliull S lIu111 2 + nuJII 2 = (u1 + u, u)
S; UuT + utll Iutt for all u e X.
This implies lIu111  2- 1 11u1 + utll. Since lIuTIl = lIu;1I and X* IS
strictly convex, IIf = ute
(II) We show that A: X -+ X* is demicontinuous. Let
u" -+ u in X
as n -+ co.
Hence IIAr4,,1I = lIu.1I -. lIuli as n  00. Since (AI4,,) is bounded and X.
is reflexive, there exists a subsequence, again denoted by (u.), such that
Au u. in X.
II
as n -+ 00.
For all v EX,
(u*, v) = lim (Au", v)  lim 1114,,11 II vII = lIuli Hvll.
II.. CIC
n-oo
Moreover,
(14.,14) = lim (All", un) = lim lIu,,)l2 = lIull 2 .
.-00
...
Hence u. = Au. The convergence principle (Proposition 21.23(i» shows
that the entire sequence (Au n ) converges weakly to u. = Au.
(III) We prove that
I . 2-lllu + lhll 2 - 2- 1 11u1l 2 _ (A ' )
1m - U"J
,o t
for all hEX. (42)
I ndeed, for all u, v EX,
(Av, v - u)  II vII 2 - IIvll nun
> IIvB 2 - 2- 1 (lIuIl 2 + IIv1l 2 )
= 2- 1 II vII 2 - 2- l lIull 2 > lIullllvll - lIull 2
> (Au, v - u).
Letting v = u + rh, hEX, l E 01, we obtain
t(AII, h) < 2- l liu + thll 2 - 2- 1 11u1l 2  t(A(u + th), h).
This implies (42), since (A(II + th), h) ..... (Ar4, h) as t -. 0 by (II).
(IV) It follows from (42) that Au = </,'(14). By Proposition 32.13(a), ccp = cp'.
Hence J = A.

864
32. Maximal Monotone Mappings
(V) Since lIuli = (2q>(U))1/2, it follows from the chain rule in Section 4.3 that
u t--+ II u II is G-difTerentiable on X - {O}.
(VI) Since qJ is even, the G-derivative J = cp' is odd. From (Ju,u) = lIull 2
and IIJull = lIull, it follows that J is coercive and bounded.
(VII) J is monotone. Indeed, for all u, v E X,
(Ju - Jv, u - v) = (Ju, u) + (Jv, v) - (Ju, l') - (Jv, u)
> lIull 2 + IIvll 2 - 2l1ullllvll.
Hence
< J u - J v, u - v) > (II U 11 2 - II V 11 2 )2
for all u, v EX.
(43)
(VIII) Since J: X -. X. is monotone, coercive, and demicontinuous, it follows
from Theorem 26.A that J is surjective.
(IX) By Proposition 32.7, J is maximal monotone.
PROOF OF PROPOSITION 32.22(b). Suppose that X is strictly convex.
(X) We show that J: X -+ X. is strictly monotone. From
(Ju - Jv, u - v) = 0
and (43) we obtain
0= \JU - J( U; V ), ".; v ) + \J( ; v ) - Jv, " 2 )
> (11"" - ";V Y +( U; -IIVIIY.
Hence lIuli = 112- 1 (u + v)1I = !lvll. Since X is strictly convex, u = v.
Consequently, J is strictly monotone.
(XI) Since the operator J: X ..... X. is strictly monotone and surjective, it is
also bijecti ve.
(XII) We show that J- 1 : X. -+ X is the duality map of X.. By hypothesis,
X is reflexive, i.e., X.. = X. Let
J: X. -+ X
denote the duality map of X.. By Proposition 32.22(a), the operator J
is characterized through
(iu.,u.)x. = lIu*'1 2 and IIJu." = "u.lI,
for all u. E X.. Since (u,u.)x. = (u.,u)x for all u E X, u. E X., it
follows from (39.) that Ju. = J-l(U.). Hence J- 1 = J.
(XIII) Let
(Ju n - Ju, Un - u) ..... 0
as n -. 00.
We want to show that Un  u in X as n ..... 00. A simple calculation shows

32.3. TYPical Examples for Maximal Monotone Mappings
865
that
(Ju" - Ju,u" - u) = (lIu,,1I - IIul1)2 + (lIu"lIlIuli - (Ju",u»)
+ (II u" 1111 u II - (J u, u" ) ).
Since each of the three terms on the right-hand side is nonnegative, we
obtain
II u" II -+ II u II
and
(Ju, u,,) -+ II ull 2
as n --. 00.
Since X is reflexive, there exists a subsequence, again denoted by (u,,),
such that
u"  v
as n -+ oc.
(44)
We have to show that u = l'. Then we are done by using the conver-
gence principle (Proposition 21.23(i)). Indeed, by (44),
(Ju, u,,) -+ (Ju, v) as n --. oc.
This implies (J u , v) = II U 11 2 , i.e., 1/ J u II II v II > II 1411 2 . Hence II vII > II u II.
On the other hand,
IIl'1I < lim Ilu,,1I = lIull.
" - oc'
Hence
( J u, (' > X = II u " 2 and Ill'" = II J u II .
By (XII), I' = J(Ju). Since j = J -1, I' = u.
If, in addition, X is locally uniformly convex, then it follows from
U"  u and lIu,,11  Hull as n -+ oc
that U" -+ u as n -+ oc: (cf. Proposition 21.23(d)).
PROOf OF PROPOSITION 32.22(c). Suppose that X. is locally uniformly convex.
(XIV) We want to show that J: X ..... X. is continuous. To this end, let
U" -+ u in X
as n -+ 00.
This implies IIJu"II -+ IIJull as n -+ 00. By Proposition 32.22(a), J is
demicontinuous. Hence
Ju"  Ju
as n -.... ex).
Since X is locally uniformly convex, Ju" -+ Ju as n ..... 'X.J.
(XV) Since J: X -+ X. is continuous and J = cp', we obtain from Proposition
4.8(c) that the G-derivative cp' is actually an F -derivative. By the chain
rule in Section 4.3, it follQws from IIuli = (2cp(U))1/2 that the norm
u....... "u II is F -d ifTeren t ia hie on X - {O}.
PROOF 01-" PROPOSITION 32.22(d). This follows from (b) and (c).

866
32. Maximal Monotone Mappings
PROOF OF PROPOSITION 32.22(e). Let X. be uniformly convex. We want to
show that J: X .... X. is uniformly continuous on bounded sets.
Let S = {u e X: lIuli = I}. We first show that J is uniformly continuous on
S. Othenvise, there exist an I: > 0 and two sequences (14,,) and (v,,) \\'ith lIu,,1I =
IIv.1I = 1 for all n such that
lIu" - v,,11 -+ 0
For all 14, v E X..
as n -+ 00
and
IIJu" - Jv,,11 > e
for all n.
]lJu + Jvllilull > (Ju + Jv, u)
= (Ju,u) + (Jv,v) + (Jv,u - v)
> Uult 1 + II vII 2 - IIvll "14 - vII.
Letting u = Un and v = v n , we get
112 -I (J 14.. + J V,.) II  I - 2 -1 tlu" - v .11.
This contradicts the uniform convexity of X*. In this connection, note that
IIJu.1I = IIJv,,1I = I for all n.
No\v observe that J(l,,') = jJ", for all W E X, ). > O. From
IIJu - Jvll = IIlIuIlJ(lIull- 1 u) - IIvIIJ(lIvll- 1 v) II
 lIullIlJ(UuR -1 u) - J(lIvlI- 1 v) II
+ 1111411 - IIvll' IIJ(lIvll- 1 v)tI,
and from the uniform continuity of J on S, it follows that J is uniformly
continuous on bounded sets that lie outside some neighborhood of the point
u = O. Hence, J is uniformly continuous on each bounded set, since J is
continuous at II = 0 and J(O) = O.
If we set t/J(u) = 111411, and tp(u) = 2- 1 I1uIl 2 , then .p(u) = (2tp(U»1/2 and tp'(u) =
Ju for each 14 e X. By the chain rule,
""(u) = l:u1
Hence the F -derivative .p' is uniformly continuous on each subset of X which
lies outside some neighborhood of the point 14 = O.
The proof of Proposition 32.22 is complete. 0
for all u #- O.
32.4. The Main Theorem on Pseudomonotone
Perturbations of Maximal Monotone
Mappings
Our objective is to solve the basic equation
b e All + Bu,
II e C,
(45)

32.4. The Main Theorem on Pseudomono(one Perturbations
867
where A: C c X  2 x . is maximal monotone and B: C -+ X. is pseudomono-
tone. As we shall show in the next sections, equations of type (45) allow many
applications. Explicitly, equation (45) means the following. For given b EX.,
we seek a u E C such that
b = l' + ",',
where l' E Au and W E Bu.
If A and B are single-valued, then (45) is equivalent to the operator equation
b = Au + Bu, u E C.
We assume:
(H I) C is a nonempty closed convex set in the real renexive B-space X.
(H2) The mapping A: C ..... 2 x . is maximal monotone.
(H3) The "mapping B: C -+ X. is pseudomonotone, bounded, and demi-
continuous.
Recall that B: C -+ X. is called pseudomonotone iff the following
holds true. For each sequence (un) in C, it follows from Un  u as n -+ 00
and
lim (Bu"t Un - u) < 0
"c.(i
that
(Bu, u - v) < lim (Bull' UtI - t,>
for all l' E C.
n-oo
(H4) If the set C is unbounded, then the operator B is A-coercive with respect
to the fixed element b E X., i.e., there exists a point Uo E C 11 D(A) and
a number r > 0 such that
< Bu, u - u o ) > < b, u - u o )
for all u E C with II ull > r. (46)
Theorem 32.A (Browder (1968)). Let b E X. be given and assume (H I) through
(H4). Then the original problem (45) has a solution.
This theorem represents a fundamental result in the theory of monotone
operators. In Problem 32.4 we will show that this theorem remains true if
B: C -+ 2 x . is multivalued. In this connection, we will use a regulariza-
tion argument. Before proving Theorem 32.A let us prove some important
corollaries.
Corollary 32.25. Suppose that (HI) through (H3) hold, and suppose that one
of the lollo\\'ing two conditions is satisfied:
(i) C is bounded.
(ii) C is unbounded, and B is A-coercit'e, i.e., there is a U o E C n D(A) such that
< Bu, u - u o )
- -- -+ + 00
II u II
as lIull -+ 00 in C.
(47)
Then, R(A + B) = X.. That is, for each b E X., the original equation b E
Au + Bu, u E C, has a solution.

868
32. Maximal Monotone Mappings
PROOF. For each b E X., the assumption (H4) above is satisfied.
o
Corollary 32.26. Suppose that:
(i) The mapping A: X -. 2 x . IS maximal monotone on the real reflexive
B-space X.
(ii) The operator B: X -+ X. is monotone, hemicontinuous, and bounded.
(iii) B is A-coercive, i.e., there exists a U o E D(A) such that
lim 5 8u . u _ u o ) = +00.
IIIIU oo lIuli
Then, R(A + B) = X*.
PROOF. By Proposition 27.6, the operator B is pseudomonotone. The assertion
now follows from Corollary 32.25. 0
We now study the unperturbed equation
bEAu,
U E C.
(48)
Corollary 32.27. Suppose that:
(i) C;s a nonempty closed convex subset of the real reflexive B-space X.
(ii) The nlapp;ng A: C -. 2 x . is maximal monotolle.
(iii) If the set C ;s unbounded, then the operator A is coercive with respect to
the fi.ed element b E X., i.e., there exist a U o E D(A) and an r > 0 such that
(u.,u - u o ) > (b,u - u o )
Tllen, equatio'J (48) has a solutio'i.
for all (u, u.) E A with IIuli > r.
PROOF. We use a regularization method. In place of (48), we study the
regularized equations
bEAu" + eIlJ(u,. - u o ), utI E C, n = 1,2,..., (49)
where J: X -+ 2 x . denotes the duality map J: X --. X., and (e,.) is a sequence
of positive real numbers with Ell -. 0 as n -. 00. According to Proposition
32.23, we introduce an equivalent norm on X such that both X and X. are
locally uniformly convex. By Corollary 32.24, the duality map J: X -. X. is
single-valued, continuous, bounded, coercive, and strictly monotone. Notice
that A remains maximal monotone and coercive with respect to b, when
passing to equivalent norms.
(I) Solution of the regularized equation (49). From (J(u - u o ), u - u o > =
II u - Uo 11 2 it follows that
I ' (J (u - u o ), u - Uo >
1m - = +00.
,Iu II -. XI II u II
According to Corollary 32.26, for each II, equation (49) has a solution U,..

32.4. The Main Theorem on Pseudomonotone Perturbations
869
(II) A priori estimates. By (49) for each  there exists a u: E A14" such that
b = u: + £n J (14" - uo).
(SO)
Hence
(b,u" - uo) = (u:,u lt - u o ) + £II(J(U" - uo),u" - "0)
= (u:, Un - Uo) + tIt II u" - Uo 11 1 (5 I )
and (u li . u:) e A for all n. By hypothesis (iii), the sequence (u II ) is bounded.
(III) Weak convergence of the regularization Inethod. Since (un) is bounded,
there exists a subsequence, again denoted by (u,,), such that
U u
n
as n -. 00.
Hence u e C.
Since A is monotone, it follows from (u II , u:) e A that
(u: - v., U. - v) > 0 for all (v, VIII) E A.
By (50),
(b - t"J(u n - uo) - v.,u" - v)  0
and all II. Notice that
lIe,.J(u n - Ilo)U = £" lIu" - Uo II ..... 0 as n.-. 00.
Letting n .-. OC'. it follows from (52) that
(b-V.,14-V» 0 forall (v,v.)eA.
for all (v, VIII) E A (52)
Since A is maximal monotone. beAu.
o
PROOF OF THEOREM 32.A. The idea of proof is the following:
(a) We use a Galerkin method. In contrast to the proof of the main theorem
on monotone operators (Theorem 26.A), we now work with Galerkin
inequalit ies.
(b) The Galerkin inequalities are solved by means of the existence principle
for inequalities in (Jilt (Proposition 2.17). In this connection, we use a
truncation method.
(c) Coerciveness implies a priori estimates for the solutions of the Galerkin
inequalities.
(d) The convergence of the Galerkin method is based on the pseudomono-
tonicity of the operator B.
Since A: C -+ 2 x . is maximal monotone, the mapping A is not empty, i.e.,
there exists a (uo, u) E A. Without loss of generality, we may assume that
Uo = 0 and u = 0, i.e.,
(0,0) E A.
Otherwise, we pass from the equation bEAu + Bu, u e C, to the modified

870
32. Maximal Monotone Mappings
equation
b - u e Av + Bv , v E C - uo,
where we set Av = A(v + flo) and Bv = B(v + uo) - u3 for all v E C - 110'
Step I: Equivalent variational inequality.
We seek a U E C such that
(b-BU-t'.'u-v)xO forall (v,v*)eA. (53)
This problem is equivalent to the original problem (45). Indeed, 14 E C is a
solution of (53) iff b - Bu e All, since A is maximal monotone.
Step 2: A priori estimates.
Let u be a solution of (53). Since (0,0) e A, we obtain
(b - Bu, u)  O.
By the coerc;ve'Jess condition (46) with Uo = 0, we get "f4n < r for fixed r > O.
Step 3: The Galerkin inequalities.
Let !£ denote the set of all finite-dimensional subspaces Y of the original
B-space X, i.e.,
Y e !i' implies Y c: X and dim Y < a::;.
We fix Ye!i'. In place of the variational inequality (53), we consider the
apprOXinJale problem
(b - BUr - v*, Uy - v)r  0, (54)
for all (v, v*) e A with v E C r. 1': where we seek "r E C n Y.
In this connection, note that X. C Y.. This is to be understood in the sense
of
(u.,U)r = (u.,u)x
for all (u*,u) e X. x y:
Step 4: Solution of (54) by a truncation method.
(IV-I) The truncated problem. Let R > O. For fixed Y e fR, we replace (54) by
the truncated problem
(b - BUR - v., U R - v>r  0 for all (v, v*) e G R (55)
and unknown Ult e Kit. Here, we set
K R := {VE C () Y: IIvll  R},
G R = {(v, v.) e A: ve K R }.
(IV-2) Solution of (55) via the existence principle for finite-dimensional t"Dr;a-
tional inequalities. By Proposition 2.17, problem (55) has a solution
UR e K R .
(IV-3) Solution of (54) via the finite intersection property. We fix Ye!fJ. Let
.9'1t denote the set of all the solutions UR e K R of (55). i.e.,
9'1t £ K It for all R > O.

32.4. The Main Theorem on Pseudomon010ne Per1urbations
871
As in Step 2 above, we obtain the a priori estimate
IIURII  r for all UR e 9'« and all R > 0,
where r is independent of R and  Since B is demicontilJuous, the set
R is closed. Moreover, .9'R lies in the compact set
{u e C '"' Y: Hun  r}.
If r  R  R', then G« s; G R ,. Hence
.9 R  9'«, for all R.. R' with R' > R  r.
Therefore, by the finite intersection principle Al (12g), there exists an
clement U)O such that
Ilr E n sPit.
Rr
Obviously, this element U r is a solution of our approximate problem
(54). In addition. we obtain that
lIu r l1 < rand "r e C " Y. (56)
Step 5: Convergence of the Galerkin method via the finite intersection
principle.
F.or each Y E !R, the approximate problem (54) has a solution Uy. We \vant
to show that (ur) "convergcs" in some sense to a solution U of the original
problem (53). To this end, \ve will use the maximal monotonicity of A and the
pseudomonotonicity of B. Since the defintion of pseudo mono tonicity is based
on sequences and not on more general M-S sequences, we need an additional
approximation argument, which will be used in (V-3) below.
(V -1) The /illite intersectiolJ prillciple. Let Y, Z E 2'. We set
f..lz = {(ur- BUr) E X x X.: "r is a solution of (54) with Y => Z}.
We want to show that there exists an ordered pair (II, u*) such that
(u_ 14-) e n J\fz , (57)
Z f: !I'
\vhere M z denotes the closure of M z with respect to the weak topology
on the product space X x X.. Furthermore, we shall show below that
II is the desired solution of the original problem (53).
We now prove (57). According to (56) there exists a closed ball K in
X x X. such that
U M z £ K.
Ze
In this connection, note that the operator B is bounded.
Since X is rejlexit. so are X. and X x X.. Consequently, K is
weakly compact (see AJ(38». Since M z is a weakly closed subset of the
weakly compact set K, the set M z is weakly compact and
U M z  K.
zc!/'

872
32. Maximal Monotone Mappings
Let Y, Z e 1£ and set S = span {  Z}. Then
Myn M z ;2 Ms.
.fhis implies
M - r n M z :F 0
for all 1': Z E IR.
The same argument shows that the intersection offinitely many sets A-/ r
is not empty, i.e.,
JW r , " ... ('\ M r " #: 0 for all f. , . . .,  E !fl.
By the finite intersection principle A 1 (12g), there exists a (u,u*) such
that (57) holds.
(V-2) Construction of a special ordered pair via Ille maximal monotonicit)' of
A. There exists a (vo, v) e A such that
(b - u* - v,u - vo>x s O. (58)
Otherwise. we have
(b -,,* - v.,u - v)x > 0 for aU (v,v*) e A.
This implies b - u* e Au, because A is maximal monotone. Letting
v = u and v. = b - u*, we get (v, v*) e A and (b - u. - v*, u - v)x =
O. This is a contradiction.
(V-3) A special approximation argument. We fix Y E fe. The s et JV y is weakly
closed in the B-space X x X*, and we have (u, u.) E My, according
to (57). It follows from Problem 32.1 that there exists a sequence of
elements (14", u:) in JW r such that
(u,., u:)(u. u*) in X x X.
as n -+ cc.
By construction of M)I,,,: = Bu". Consequently, there exists a sequence
(u.) in C such that
UIIU in X
and
Bu u* in X*
n
as n -. 00 (59)
and
( b - Bu - v* u - V )r > 0
" ," -
for all (v t v*) E A with v e 1':
(60)
according to (54). Since C is closed and convex. we obtain
14 e C.
(V-4) Pseudonlonotonicity of B. We want to show that
(Bu, u - v)  (v* - b, v - u) for all (v, v*) E A with v e y: (61)
In the following proof of (61 we consider only such Y e!l' with
Vo e y, where the fixed element Vo satisfies (58). Notice that
u y = X.
r it Y.r.o r
(62)

32.5. Apphcatlon to Abstract Hammerslein Equations
873
We now fix Y E Y. From (60) it follows that
(Bu", u" - "-') < (I'. - b, v - u,,) + (Bull' I' - "'), (63)
for all \\' E C.. (l', v.) E A and n E N. Letting \V = (1, we obtain
( 8u Il - I' ) < ( l'. - b I' - U )
II' " - , "
for a II ( l" v.) E A wit hI' E Y,
(64)
and for all n E N. Choosing w = u, v = Va, and (,. = t' in (63), we get
lim (Bu", U" - u) < (t' - b + u., l'o - u),
II-J:
since BUll  u. as n -+ 00.
By (58), (l' - b + u., V o - u) < 0 and hence
lim (Bu", u" - u) < o.
"-XI
Moreover, we have u"  u as n -+ ,XI. Since B: C -+ X. is pseudomono-
tOile.. we obtain
(Bu, II - v) < lim (Bu", Il" - I')
for all I' E C.
(65)
" - -x;
Now, relation (64) implies (61).
(V-5) Solution of the original problem (53). From (61) and (62), it follows that
(Bu, u - l') < (v. - h, I' - u) for all (I', v.) E A,
i.e... u is a solution of (53).
This completes the proof of Theorem 32.A.
o
32.5. Application to Abstract Hammerstein Equations
We consider the equation
u + KFu = 0,
U EX..
(66)
Theorem 32.8. Suppose that:
(i) The operator K: X -+ X. is linear and nZ01lotone on the real reflexive
B-space X.
(ii) The operator F: X. -+ X is pseudomonotone, bounded, and coercive.
Then, equation (66) has a solution, and this solution is unique if one of the
follo"';ng t\\'O additional conditions is satisfied:
(a) F is strictly monotone.
(b) K is strictly monotone, and F is monotone.
If X is an H-space, then we can replace assumption (ii) with the following
condition: The operator F: X. -+ X is monotone, hemicontinuous, and 3.-

874
32. Maximal Monotone Mappings
monotone. This follows from Theorem 32.0 in Section 32.22. The advantage
of this modification is that F need not be coercive. In Section 32.21 we show
that large classes of monotone operators are 3*-monotone (e.g., monotone
Ncmyckii operators on Lz(G) are 3*-monotone in the case where G is a
bounded region).
PROOF.
(I) Existence. Problem (66) is equivalent to the equation
OEK-IU+Fu ueX..
(67)
By Proposition 26.4, K is continuous, and according to Propositions 32.5
and 32.7, the mappings K and K- I are maximal monotone. Moreover,
by Proposition 27.7, F is demicontinuous. Now, it follows from Corollary
32.25 that equation (67) has a solution.
(II) Uniqueness. Let
u. + KFu. = 0
I I'
Ui eX",
; = 1, 2.
(68)
Ad(a). Since K is monotone, it follows that
(14 1 - u2,Fu l - FU2) = -(KFu. - KFzu,f.u 1 - f""2) SO.
Hence "I = "2' according to the strict monotonicity of F.
Ad(b). Since F is monotone, it follows from (68) that
o S (u, - "2' FU 1 - FU2) = - (KFu l - KFu2' Fu , - FU2)'
Because of the strict monotonicity of K, we obtain f.u 1 = FU2' By (68
III = U1' 0
32.6. Application to Hammerstein Integral Equations
Theorem 32.8 allows applications to Hammcrstein integral equations
Il(X) + fG k(x,y)f(y,ll(y»dy = 0 on G, "E L.(G),
where G is a bounded region in RN and I < q < 00. Such applications can be
found in Proposition 28.4 in connection with other existence results for
Hammerstein integral equations.
32.7. Application to Elliptic Variational Inequalities
By an elliptic variational inequality, we understand the following problem.
For given bE X., we seck a u e C such that
(b - A 14, U - v)  0
for aU v e C.
(69)

32.7. Application to Elliptic Variational Inequalities
875
By means of the indicator function X. of C, problem (69) can be written in the
equivalent form
b E ex. + Au,
U E C,
(70)
where we use the subgradient ex. from Example 32.15.
Theorem 32.C. Suppose that:
(i) C is a nO'Jempty closed C011l'eX subset of the real reflexive B-space X.
(ii) The operator A: C ..... X. is pseudomonotone, demicontinuous, and bounded.
(iii) In the case where C is unbounded, there exists a point U o E C such that
( Au, u - u o )
IIuli -+ +00
as lIull -+ oc; in C.
Then the following hold true:
(a) Existence. For each b E X., the variational inequality (69) has a solution
u E C.
(b) Solution set. If A: C ..... X. ;s monotone, then the set of all the solutions
II E C of (69) is closed and conL'ex.
(c) Uniqueness. ,r A: C -+ X. ;s strictly monotone, then the solution u E C of
(69) is unique.
Corollary 32.28. Assumption (ii) holds true in the case where one of the folioK'ing
t",.o conditions is satisfied:
() The operator A: X -+ X. is monotone, hemicontinuol4s, and hounded.
(p) The operator A: X ..... X. is pseudomonto1Je and bounded.
Variational inequalities and their numerous applications will be studied in
greater detail in Parts III through V.
PR()()F. Ad(a). By Example 32.15, the mapping aX.: C ..... 2 X . is maximal
monotone, and t7x(uo) =1= 0. Corollary 32.25 yields the assertion.
Ad(b). Let A: C..... X. be monotone. Then, the original problem (69) is
equivalent to
(b - Av, u - v) > 0
for all v E C,
(71 )
where we seek U E C. Indeed, if u E C is a solution of (69), then
(AV,l' - u) = (Au, v - u) + (Av - Au,v - u)
> (Au,v - u) > (b,v - u) for all v E C,
i.e., u is a solution of (71). Conversely, let u E C be a solution of (71). We set
('=(I-t)u+t, O<t<l, WECo
Since C is convex, [' E C. By (7 J),
(b - A(u + t(,,' - u)),u - ",) > 0
for all ,'" E C.
As t -+ + 0, we obtain (69), since A is demicontinuous.

876
32. Maximal Monotone Mappings
Let Y denote the set of all the solutions u e C of (71). From (71) it follows
that
U, II E !/
implies
(I - t)11 + Iii E .9
for 0 < t < 1,
and
u. e 9'
for all n
and
lim U II = U
implies
U E f/.
II  :(1
Thus, /f is convex and closed.
Ad(c). Let u and u be solutions of (69). i.e., for all v E C,
(b-Au,u-t,'» O and (b-A rl,u -v» O.
Letting v = u and v ::: U, we obtain
(All - Au, II - ii) S O.
Since A is strictly monotone, u = u .
o
Corollary 32.28 follows from Section 27.2.
32.8. Application to First-Order Evolution Equations
We study the initial value problem
u'(t) + Au(t) = b(t
u(O) = 0,
O<t<'1:
(72*)
and the periodic problem
u'(I) + AI4(t)= b(t), 0 < t < 1:
11(0) = u(T).
In order to obtain the corresponding operator equations, we set
Ltu = u', D(L t ) = {u e W p 1 (0, T; V, H): u(O) = O},
L 2 u = 14', D(L 2 ) = {u E Wi (0, T; V, H): u(O) = u(T)}.
We now replace (72*) and (73-) with the operator equation
L 1 u + Au = b, u E D(L 1 ), (72)
(73*)
and
L2.u + Au = b,
u e D(L 2 ),
(73)
respectively.
Theorem 32.D. LeI "V  H  V. 99 be an evolutioll triple, and leI
X = Lp(O, T; V), ",here 1 < p < 00 and 0 < T < 00. SlIppose that the

32.9. Application to Time-Periodic Solutions
877
operator
A: X  X.
is pselldol1JolJotolJe, coercil:-e, and bOllnded.
Then, for each b e X., problems (72) and (73) have soilltions. If, in addition,
A is stricti}' monotone, thell tile correspoIJding .olutioIlS are ulliqlle.
PROOf.
(I) Existence. By Proposition 32.10, the operators L j : D(LJ)  X -+ X.,
j = 19 2, are maximal monotone. The assertion then follows from
Corollary 32.25 with C = X and U o = o.
(II) Uniqueness. Since LJ is monotone, it follows from
Lj111c + Aurc = b, j, k = 1, 2,
that
0= (Lj(rI 1 - "2),U 1 -112) + (Au! - AU2,11! - "2)
 (Au. - AU2, u 1 - u 2 ).
Since A is strictly monotone.". = 112. 0
32.9. Application to Time-Periodic Solutions for
Quasi-Linear Parabolic Differential Equations
Let QT = G x ]0, T[. We consider the time-periodic problem
u,(x, t) + Lu(x, t) = f(x, t) on Qr,
DlJ u (:(, t) = 0 on aG x [0, T] for all p: IPI < m - 1, (74.)
u(., 0) = u(x, T) on G,
\vherc
Lu(, t) = L (- I  1)2 AGr(.' Du(x, I), t)
lot Slit
and Du = (DIJ u"1 ..' i.e., for each fixed t, the differential operator L is of order
2na with respect to the spatial \'ariable x, where nl = I, 2, ... . Note that the
derivatives D tI and DIJ refer to x. The condition "u(x,O) = u(x, T) on G' means
that we are looking for solutions of time-period T.
We want to replace this problem with the generalized problem
II' + All = b, u(O) = u(T), u e W"I(O, T;  H). (74)
To this end, we make the following assumptions:
(AI) G is a bounded region in (RH, N > 1. Let 0 < T < cc, 2 < p < 00, and
q-l + p-I = I. We set
v = W,"'(G),
H ::. L 2 (G),
X = L,(O, T; V).

878
32. Maximal Monotone Mappings
(A2) Let Ie L.(QT) be given.
(A3) For each fixed I e ]0, T[, the differential operator L satisfies the assump-
tions (HI) through (H4) of Proposition 26.12 (Caratheodory condition,
growth condition, monotonicity, and coerciveness). In this connection,
the constants and the functions 9 and h that appear in (H 1) through (H4)
should be independellt of time t. Moreoever, the real function
,.-. f. L As(x. Du(x), t)D«v(x)dx
G lilt m
is measurable on ]0, T[ for all u, v e J':
By Section 30.4, there exists an operator A(r): V ... V* such that
(A(t)u, v)y = f. L A(x,Du(x), t)DClv(x)dx,
G I Sift
for all II, v e  t E ]0, T[. Letting w = Au. where wet) ::: A(t)u(t we obtain an
operator
A: X -+ X.,
which is monotone, hemicontinuous, bounded, and coercive, according to
Section 30.4 and Theorem JO.A(c). Moreover, there exists a functional b e X*
such that
(b(t),v).. = fG!(X,t)V(X)dX
for all v e 
Proposition 32.29. Under the assumptions (A I) through (A3), tI,e generalized
problenl (74) corresponding to (74*) has a solution. If A is strictl)7 monotone,
then the solution is unique.
PROOF. This follows from Theorem 32.D.
o
Let m = 1. As a special case of (74*), we consider the following problem:
N
u,(x, t) - L D,(IDtu(x, 1)1,,-2 D.u(x, t) :::: f(x, t) on QTt
'=1
u(x, t) = 0 on oG x [0, T],
u(x,O) = u(x, T) on G,
where x = ('I'...' N) and D, = O/O,.
(75)
Let G be a bounded region in R N , N  1, and let 0 < T < 00, 2  p < c(),
and q-l + p-l = 1. Set
V = W p 1 (G), H = L 2 (G), X = L,,(O, T; V).
By Proposition 26.10, the assumptions of Proposition 32.29 are fulfilled.
In addition, the operator A: X -+ X. is uniformly monotone, according to

32.10. Application to Second-Order Evolution Equations
879
Corollary 30.12. Explicitly, we have
f. '
(A(t)lI, v)v = L ID,u(x)I,,-2 D.u(x)D,v(x)dx,
G '=1
for aU II, v E It:
According to Proposition 32.29, for each given Ie L,(QT)' the generalized
problem (74) corresponding to (75) has a unique solution.
32.10. Application to Second-Order
Evolution Equations
We now study the following initial value problem:
u ll + Nu' + Lu = b,
u(O) = u'(O) = 0,
U E C([O, T], V u' e W p 1 (0, T;  H).
(76)
We set
x = L,(O, T; V).
Then X. = Lq(O. T; V.), where p-l + q-l = 1. If 2 S P < 00, then q  p and
hence X s: X*. The condition u' e W,:(O, T; H) means that
u' e X and UN eX..
Let u' e W p 1 (O, T; V,H). After changing the function I.....U'(t) on a subset of
]0. T[ of measure zero. we obtain a uniquely determined function
u' e C([O. T], H),
i.e., u': [0, T] ... H is continuous. The initial condition u'(O) = 0 above is to
be understood in the sense of u' e C([O, T],H). We assume:
(HI) Let 2  p < 00 and 0 < T < 00, and let "V s H s v*n be an evolution
triple.
(H2) The operator N: X -+ X. is monotone, hemicontinuous, coercive, and
bounded.
(H3) The operator L: V -+ V. is linear, monotone, and symmetric, i.e.,
(Lv, w)y = (Lw, v)y for all v, W e J1:
For u e X, we set (LU)(I) = Lu(t).
Theorem 32.E. Under the assumptions(Hl) through (H3),lor each given be X.,
the initial value problem (76) has a solution. If N is strictly monotone, then this
solution is unique.
Applications of this theorem to nonlinear hyperbolic differential equations
will be considered in the next chapter.

880
32. Maximal Monotone Mappings
The idea of proof is to introduce the operator S by
(Sv)(t) = f v(s)ds.
Letting u = Sv, the original problem (76) is reduced to the first-order evolution
equation
v' + Nv + LSv = b. v E W,l(O. T; V, H),
v(O) = o.
(77)
PROOF.
(I) Equivalence of (76) and (77). Let f4 be a solution of (76). We set
I' = u'
and
\\. = Sv.
From ve C([O, T], H) it follows that ", E C 1 ([0, T], H). This implies
\V' = v. Since u(O) = ,,'(0) = 0, we obtain ,,,. = u. Hence, v is a solution
of (77).
Conversely, let v be a solution oC(77). Hence ve C([O, T]; H). We set
14 = Sv.
Thus. 14' = v. Since v E L,(O, T; V), we obtain u e C([O, T], V). Conse-
quently, u is a solution of (76).
(II) We want to show that the operator LS: X  X. is linear and
continuous.
(II-I) The operator S: X -+ X is continuous. Indeed, for all t' e X, the Holder
inequality implies
IISvU = f: I f v(s)ds " dt
 T(foT II v(s) II ds r
 const f: Uv(s)II'ds = const IIv!l.
(11-2) By assumption, thc operator L: V -+ V. is linear and monotonc. By
Proposition 26.4, L: V -+ V. is continuous. Thus, for all veX, we
obtain
IILt'II. = f: IILv(s)II. ds
const f: IIv(s)lIt-ds
(J. T ) tlIP
 const 0 IIv(s)lI ds = const IIvlli.
since I < q  p. Hence L: X -+ X. is linear and continuous.

32.11. Regularization of Maximal tonotone Operators
881
(III) The operator LS: X -+ X* is monotone. To prove this, let P denote the
set of all polynomials p: [0, T] -. V with p(O) = 0, where the coefficients
of p live in Jt: Since P is dense in X" it is sufficient to show that
<LSp,p>xO forall peP. (78)
Let q e P. Using the product rule and the symmetr}' of L: V -. V*, we
obtain
d
dl (Lq(t). q(l»., = (Lq'(t), q(t»... + (Lq(t), q'(t) >.,
= 2<Lq(lq'(t»v,
and hence
2 f (Lq(s), q'(s»., ds = (Lq(t), q(l».,  O. (79)
for aliI E [0, T]. For q = Sp, pEP, this means
2 f (L(Sp)(s), p(s»., ds > O. (79*)
Letting t = T we get (78).
(IV) Existence. Since LS: X ..... X. is linear, continuous and monotone the
operator
N + LS: X ..... X.
is monotone, hemicontinuous. coercive, and bounded. By Theorem
32.D, the initial value problem (77) has a solution.
(V) Uniqueness. If N: X -+ X. is strictly monotone, then so is N + LS.
According to Theorem 32.D, the solution of (77) is unique. 0
Since P is dense in X, it follows from (79*) that
f (L(Sv)(s v(s»v ds  0 (80)
for all v E X and all t E [0, T]. This inequality will be used in the proof of
Theorem 33.A.
32.11. Regularization of Maximal
Monotone Operators
The following theorem characteri7.cs maximal monotone operators in terms
of the duality map.
Deorem 32.F (Rockafellar (1970). Let X be a real reflexive B-space, where X

882
32.. Maximal Monotone Mappings
a,ul X. are strictly COli vex. Tilell the monotone mapping
.
A: X -. 2 x
is maxinlal mO'lotone iff R(A + J) = X..
Here. J: X -. X. denotes the duality map of the space X.
CoroUar)' 32.30 (Regularization), Let C be a nonempt}' closed convex subset of
the real reflex;t,'e B-space X, \\Jhere X and X. are stricti)' convex. Suppose that
tile mapping A: C -. 2 x . is maximal monotone.
Then, for each A > 0, the inverse operator
(A + AJ)-I: X. -. X
is single-valued, demicontinuous. and max;n,al monotone.
PROOF OF COROLLARY 32.30. Let A > O.
(I) Surjectivity. By Proposition 32.22, the duality map J: X -. X. is
single-valued, strictly monotone, dcmicontinuous, and bounded. From
(JU,14 - 140> > UuU 2 - lIulilluo JI it follows that
I . (Ju,u-uo>
1m = +00
IluL"'«J Hull
for each Uo e X.
By Corollary 32.25, we get R(A + AJ) = X*.
(II) Single-valuedness of (A + AJ)-l. Let u. V E (A + )J)-I(b). We set
c = b - AJ u and d = b - AJ v.
Hence c e Au and d e Av. By the monotonicity of A.
o = (b - b,u - v) = (c + AJu - (d + )Jv),u - v)
= (c - d,u - v) + A(Ju - Jv,u - v)
 A(Ju - Jv,u - v).
Since J is strictly monotone, u = v.
(III) The operator A + AJ: X ..... 2 x . is monotone, since A and J are mono-
tone. This implies the monotonicity of the inverse mapping (A + )J)-I:
X* -. X. By Proposition 26.4, (A + ll)-1 is locally bounded.
(IV) The operator (A + AJ)-I: X. -. X is demicontinuous. To prove this, let
b ll -+ b in X.
as n -. 00.
We set u.. = (A + ,u)-1 bIt and u = (A + U)-l b. This implies
Au. + AJu. -. Au + AJu
as n -. 00.
Since (A + )J)-I is locally bounded, the sequence (u lI ) is bounded.

32. t 2. Regularization of Pseudomonotone Operators
883
Hence
(Au" + i..Ju" - (Au + UII),lI n - ")
= (Au. - Au, u. - u) + ).(Ju. - Ju, UII - U) -+ 0 as n -+ .
Since the two right-hand terms are nonnegative, it follows that
(Ju n - Ju, Un - u) ..... 0 as n -+ 00.
By (40 this implies Un  II as n -+ 00.
(V) According to Proposition 32.7, the monotone demicontinuous operator
(A + U)-t: X. -+ X is maximal monotone. 0
PROOF OF THEOREttt 32.F.
(1) If A: X -. 2 x . is maximal monotone, then it follows from Corollary 32.30
that R(A + J) = X*.
(II) Conversely, let A: X -+ 2 x . be monotone and suppose that R(A + J) =
X*. The proof ofCoroUary 32.30 shows that (A + J)-I: X. -+ X is mono-
tone and demicontinuous, and hence maximal monotone. By Proposition
32.5, the inverse mapping
A + J: X -+ 2 x .
is also maximal monotone. To prove the maximal monotonicity of At let
(u, u.) E X X X. and suppose that
(U. - v., II - v) > 0
for all (v, v*) e A.
This implies
(u. - (w. - Jv), u - v)  0 for all (v, w.) e A + J.
From (Ju - Jv,u - v)  0 we get
(u* + Ju - \v*, U - v)  0 for all (v, Mt.) e A + J.
Since A + J is maximal monotone, we obtain u. + Ju e (A + J)u, and
hence u. e Au. 0
32.12. Regularization of Pseudomonotone Operators
Proposition 32.31. Let X be a real reflexive B-space, where X and X. are locall)'
uniformly convex. Further let
A: C c X -+ X.
be a pseudomonotone operator on the nonempty closed convex set C.
Then, for each ,t > 0, the operator A + )J: C -+ X. satisfies condition (S)+.
PROOF. Let ). > 0, and set AA = A + )J. Suppose that u.u in C as n -+ 00,

884
32. Maximal Monotone Mappings
and
lim (A AU. - A,tu,U" - u)  O.
"  IX,.
We have to show that U"  II as n  r:£. Indeed, from the decomposition
(A AU" - AJ-U, UtI - u) = (Au" - Au, II" - II) + ;"(Ju" - Ju, u" - u) (81)
and (Ju,. - JII, U" - II) > 0, it follows that
lim (Au,. - AII,u. - u) < o. (82)
"  CI:'
Since A is pseudomonotone, this implies
(AII,u - v) S lim (Au", II" - v)
for all v E C.
" -. :JO
Letting r: = u, we obtain
lim (Al,,,, U" - II) > o.
" -. :JO
Hence
lim (Au" - Au. U II - u)  o.
(83)
,,"'a:;
By (82) and (83), lim,,-. (Au" - Au,u" - u) = O. From (81) we obtain
lim (Ju" - Ju, II" - II) = o.
n
According to Proposition 32.22. this implies u" .... u as n .... 00.
o
32.13. Local Boundedness of Monotone Mappings
Definition 32.32. Let Y and Z be B-spaces. The mapping A: Y --. 2 z is called
locall}' bounded at the point y iff there exists a neighborhood U of }' such that
t he set
A(U) = U Au
ucV
is bounded. Moreover, A is locally bounded on the set D iff A is locally
bounded at each point of D.
The local boundedness of monotone operators is a fundamental property
of these operators. The following result will be used critically in the next
section.
Proposition 32.33. A nwnOlone ,napping A: X -+ 2 x . on ti,e real a-space X is
locally bOllnded at each interior point of D(A).
PROOF. Our proof makes use of the Baire category.

32.13. Local Boundedness of Monotone Mappings
885
(I) Let (":) be a sequence in X. with
inf (u:, u> > - 00 for all u on a fixed open set.
II.U
Then
sup ,'u: II < 00.
"
(84)
Indeed, it follows first that there exist an r > 0 and a ball B =
{v EX: IIvl < r} such that
inf (":. U o + v) > -00
for aU v e B and fixed Uo.
".v
Since 0 e B, this implies inf". (u:, :t v) > -OCt for all v e B, and hence
sup I(u:, v)1 < 00 for all v e B.
11.1'
This yields (84).
(II) Let (u II ) and (u:) be sequences in X and X., respectively, such that
u" ..... 0
and
ilu: II --. 00
as n  00.
Then,. for each r > 0, there exists a veX with II vII s r such that
lim (u:,u" - v) = -00.
. ... JO
Otherwise, there exists an r > 0 such that
lim (u:, u. - v) > --00,
....00
for each v in the ball B = {VEX: IIvli  r}. This implies
rr:-
B = U B.c.
i-I
where B" = {v E B: (u:, u" - v)  - k for all n}. The set  is closed for
each k. Since the closed ball B in the B-space X has second Baire category
(cf. A 1 (65a)), there exists at least one B.c which has nonempty interior.
This implies
inf (II:, u) > -co
for all u in a small ball.
II."
By (I), the sequence (u:) is bounded in X*. This is a contradiction.
(III) We now prove the statement of Proposition 32.33 by contradiction. If A
is not locally bounded at a fixed point u e iot D(A), then there exists a
sequence of points (u II , u:) e A such that
u. -+ u
and
lIu: I -. 00
as n -to 00.
We may assume that u = 0, since the monotonicity of A is invariant
under translation. Choose an r > 0 such that the ball B = {veX: II v II < r}

886
32. Maximal Monotone Mappings
lies in D(A). According to (II), there exists a v E B such that
lim (u:, U" - v) = -00.
(85)
" ... 00
For fixed (v, v*) E A, the monotonicity of A implies
(14:, U" - v) > (t,*, U" - v)
This contradicts (85).
for all n.
D
32.14. Characterization of the Surjectivity of
Maximal Monotone Mappings
By definition of the range of A, R(A), the equation
bEAu, U E X,
has a solution for each given b E X* iff R(A) = X*.
(86)
Theorem 32.G (Browder (1968a)). Let A: X -+ 2 x . be a maximal monotone
mapping on the real reflexive B-space X. Then. R(A) = X* iff A -I: X* -+ 2 x is
locally bounded.
The proof will be given below.
In order to formulate important consequences of this theorem, we need the
following notions.
Definition 32.34. Let A: X -+ 2 x . be a mapping on the real B-space X.
(a) A is called coercil'e iff either D(A) is bounded or D(A) is unbounded and
inf". E Au (u*, u)
lIuli -+ +00
(b) A is called weakly coercive iff either D(A) is bounded or D(A) is unbounded
and
as II u II -. 00, U E D(A).
inf II u*1I -+ + 00
u. E Au
as lIuli -+ 00, u E D(A).
S i nee < u. , u)  II u .1111 u II, we have:
A is coercive implies A is weakly coercive.
In particular, let A be single-valued, i.e., we consider the operator A: D(A) c
X -+ X*. Then A is coercive iff either D(A) is bounded or D(A) is unbounded
and
(Au, u)
lIuli -+ +00
as "ull -. 00, u E D(A).

32.14. Characterization of the Surjcctivity or Maximal Monotone Mappings
887
Moreover, A is weakly coercive iff either D(A) is bounded or D(A) is un-
bounded and
IIAull -+ 00
as Ilu II ..... 00, u e D(A).
Corollary 32.35. Let A: X .... 2 x . (resp. A: D(A) c X -+ X.) be a maxinJal mono-
lone and "teakl)' coercite mapping on the real reflexive B-space X. Then
R(A) = X*.
PROOF. The weak coerciveness of A implies the local boundedness of A-I.
Theorem 32.G ylelds the assenion. 0
Theorem 32." (Main Theorem on Weakly Coercive Monotone Operators).
Let A: X -. X. be a monotone, hemicontinuous, and weakly coercive operator
on the real reflexive B-space X.
The" R(A) = X*.
PROOF. By Proposition 32.7, the operator A is maximal monotone. Corollary
32.35 yields the assertion. 0
PROOF OF THEOREM 32.G. The key to the proof is the method of regularization
in (11-3) below.
(I) Let R(A) = X*. Since A is maximal monotone, so is A -I. According to
Proposition 32.33, the mapping A-I: X. ... 2 x is locally bounded.
(11) Conversely, let A -I: X. -+ 2% be locally bounded. We want to show that
R(A) = X*. To do this. it is sufficient to prove that R(A) is nonempty,
closed, and open.
(II-I) R(A) is nonempty. Indeed, it follows from the maximal monotonicity of
A that A :F 0 and hence R(A) #; 0.
(11-2) R(A) is closed. To prove this, let u: e Ar'n for all nand
u* -. u.
.
as n -. 00.
By the monotonicity of A, we obtain
(u: - v., u. - v)  0 for all (v. v*) e A and all n. (87)
Since A -1 is locally bounded, the sequence (un) is bounded. Thus, there
exists a subsequence, again denoted by (u ll ). such that
u u
..
as n -+ 00.
From (87), we get
(u* - v. t U - v)  0
for all (v, v*) e A.
Since A is maximal monotone, this implies u. E Au, i.e., u. e R(A).
(11-3) R(A) is open. To show this, let
u. e R(A

888
32. Maximal Monotone Mappings
i.e., u* E Au for some u. Since the maximal monotonicity of A remains
invariant under a translation, we may assume that u = O. We now
choose an r > 0 such that A -1 is bounded on the ball
B = {v* E X*: IIv. - u*1I < r}.
Our goal is to show that
IIv. - u*1I < r/2
implies
v* E R(A).
(88)
This yields the openness of R(A).
In order to prove (88), we use the method of regularization. We first
equip the B-space X with an equivalent norm such that both X and X*
are strictly convex. Let (,. be given such that
II v* - u*1I < r /2.
By Corollary 32.30, for each A > 0, the equation
v! + )'Ju;. = v*,
v! E Au;.,
(89)
has a solution u A . This existence result is the key to our proof. By the
monotonicity of A, we obtain
(v. - AJuJ, - u*, UJ. - u) > 0 for all A > 0,
where u = O. This implies IIv* - u*Ullu;." - Allu;.112 > 0 and hence
lllu;.11 S IIv. - u*1I < r/2 for all A. > O.
By (89),
II v. - vf II = ,iItJu;. II = A lIu;.U < r/2,
(90)
and thus
IIv!-u*1I <r forall A>O. (91)
Since A - J is bounded on the ball B, it follows from (91) and U;. E A -I vr
that (u;.) is bounded. By (90),
1'r -+ v.
as A. -+ O.
Since R(A) is closed, we obtain v. E R(A).
o
32.15. The Sum Theorem
Theorem 32.1 (Rockafellar (1970)). Let the mappings A, B: X .-. 2 x . he maximal
monotone on the real rej1exive B-space X and let
D(A) n int D(B)  0.
V,en, the sun, A + B: X -+ 2 x . is also n,aximal monotone.

32.1 S. The Sum Theorem
889
Applications of this important result will be considered in the next three
sections.
PROOF. The main idea of our proof is to use Section 32.11 on the regularization
of maximal monotone operators (Theorem 32.F). Furthermore, we use the
main theorem on maximal monotone operators in Section 32.4 and a trunca-
tion argument based on the maximal monotonicity of subgradients.
Passing to an equivalent norm on X, we may assume that X and X. are
strictly convex. Moreover, using a translation, we may assume that
o e D(A) " int D(B).
(92)
Finally, replacing r4  Au with u t--+ Au + c for fixed c, we may assume that
o e A(O).
(93)
Step 1: Suppose that D(B) is bounded.
The mapping A + B: X ... 2 x ' is monotone. By Theorem 32.F, the mapping
A + B is maximal monotone iff R(A + B + J) = X.. Therefore, it is sufficient
to prove that, for each b* E X., the equation
b* e Au + Br4 + Ju, u E X,
has a solution. Replacing u..-. Bu with u Bu - b*, it is sufficient to prove
that the equation
o e Au + BII + )11,
U E X,
(94)
has a solution. To obtain a solution u of (94), it is sufficient to find a (14, b) such
that
-b E (A + 2- I J)u,
be (B + 2- 1 )u.
(u,b) e X x X.,
(95)
To this end, we set
Eb = -(A + 2- 1 ))-1( -b),
Fb = (8 + 2- 1 J)-I (b).
By Corollary 32.30, the operators
E, F: X. -+ X
are monotone and demicontinuous. Moreover, we have
R(F) = D(B + 2- 1 J) = D(B),
and hence
R(F) is bounded and 0 E int R(F). (96)
No\\', to solve problem (95), it is sufficient to solve the equation
Eb+Fb=O, be X.. (91)

890
32. MaximaJ Monotone Mappings
In the following we want to solve (97) by means of the main theorem on
maximal monotone operators in Section 32.4. Our considerations above show
that the solvability of(97) implies the desired maximal monotonicity of A + B.
(I) The operator E + F: X. -. X is monotone and demicontinuous. Thus,
E + F is maximal monotone.
(II) Since J(O) = 0 and hence 0 e (A + 2- 1 J)(O), we get E(O) = O. From the
monotonicity of E, it follows that
(Ev, v)  0 for all v e X*. (98a)
(III) We shall prove below that there is an r > 0 such that
(Fv, v) > 0 for all VEX. with IIvll > r. (98 b)
Adding (98a) and (98b). we get
«E + F)v, v) > 0 for all v e X* with I1vU > r,
i.e., E + F is coercive with respect to O. By the main theorem on maxi-
mal monotone mappings (Corollary 32.27), equation (97) above has a
solution.
(IV) We finish our argument by proving (98b) by means of (96). From the
monotonicity of F, it follows that <Fv - Fw, v - w) > 0 and hence
(Fv, v)  (F\v, v) + (Fv - F,,', w) for all v, w e X*. (99)
Since R(F) is bounded, there is an R > 0 such that
I(Fv - Fw, w)1  R IIwll for all v, w e X*. (100)
By Proposition 32.33, the monotone mapping F-l: X ... 2.1'. is locall)'
bounded at O. In this connection, note that 0 e int R(F). Thus, there exist
positive numbers o and e such that
IIFwll  6 0 implies IIwll  t.
Since 0 e jnt R(F), there exists a b such that 0 <  < b o and the equation
u = F\", we X,
has a solution for each given r4 e X. with lIuli  . From (99) and (100),
we obtain
(Fv, v)  sup (14, v) + (Fv - Fw, "r)
lul;6
 6 II vII - Rt
for all v E X*.
This implies (98b).
Step 2: Suppose that D(8) is unbounded.
We want to reduce this case to Step 1 by using a truncation argunJent. We
may assume that
o e D(A) n int D(B)
and
o e A(O), 0 E B(O).

32.15. The Sum Theorem
891
We set
y (u) = { o
At, + 00
jf II u" S r,
if II u" > r,
i.e., l" is the indicator function of the closed ball {II e X: lIuli S r}. For the
corresponding subgradient, we obtain
{O}
Ol,,(U) = {)Ju: 1 > O}
o
if lIuli < r,
if lIuli = r,
if lIuli > r.
In this connection, note that u. E Ox,(u) iff
x,(v)  L(ll) + (u., v - u)
for all veX.
For example, let Ilull = r. Then u. e £11,(u) iff
(u., v - u) S 0 for all v: lIvll < r.
This implies (U*,ll) = SUPIK'I='(u.,v) and hence
(u*, u) = lIu*1I r = lIu.lllluli.
By (39*), we obtain that u* e OXr(u) iff u* = Uu for some l  o.
By Proposition 32.17, the subgradient OX,,: X --. 2 x . is maximal monotone.
(I) We show that the mapping
A + (B + aX,): x -+ 2 x .
is maximal monotone for each r > O. To this end, we use Step 1.
0-1) The mappings B, aX,: x -+ 2 x . are maximal monotone and
o e D(B) " int D(oX,).
Since D(Ol,,) is bounded, it follows from Step I that B + aX,,: x -. 2 x . is
maximal monotone.
(1-2) The mappings A, B + OX,: X .... 2 x . are maximal monotone. We have
D(B + aX,,) = {u e D(B): lIuli  r}.
From 0 e D(A) () iot D(B) we get
o e D(A) n int D(B + OX,,).
Since D(B + ox,) is bounded, it follows from Step 1 that A + (B + aX,,):
x -+ 21'. is maximal monotone.
(II) We show that A + B: X .... 2 x . is maximal monotone. To this end, we
set C = A + B. By Theorem 32.F, it is sufficient to prove that
R(C + J) = X*.
(101)
Since C + OX" is maximal monotone, we have R(C + oX, + J) = X*,

892
32. Maximal Monotone Mappings
according to Theorem 32.F. Thus, for each b E X., the equation
b E Cu + ('X,(u) + Ju,
U E X,
has a solution. We choose an r > 0 such that r > IIbll. The explicit form
of Vx, yields
b E Cu + (I + )Ju,
( 102)
where lIuli $; rand
l = { o
> 0
if Ifull < "
if " u" = ,.
To prove (101), it is sufficient to show that lIull < r. Indeed, it follows
from (102) that there exists a u. E Cu such that
b = u. + (I + A)Ju,
This implies
(b,u) = (u.,u) + (I + l)(Ju,u).
Since C is monotone and 0 E C(O), we get (u., u) > o. Noting that
(Ju,u) = lIull 2 , we obtain
(b, u) > (I + A) II U 11 2 ,
and hence (I + l) II u II < II b II < r. Therefore, II u II < r.
The proof of Theorem 32.1 is complete.
o
32.16. Application to Elliptic Variational Inequalities
We want to solve the variational inequality
(Au, v - 14) > q>(u) - cp(v)
for all v EX,
( 103)
where we seek u E D(A) (\ D(otp). By definition of the subgradient ocp in Section
32.3c, this inequality is equivalent to - Au E o<p(u). Consequently, problem
(103) is equivalent to the equation
o E Au + iJcp(u),
U EX.
(104)
In particular, if cp is the indicator function of the nonempty closed convex set
C in the B-space X, i.e.,
<p(u) = { o
+00
then problem (103) is equivalent to
if u E C,
if u fI C,
(A u, v - u)  0
for all v E C,
where we seek U E C.

32.17. Application (0 Evolution Variationallnequalilies
893
In this special case, assumption (H2) below is satisfied. We assume:
(HI) The mapping A: X --.21'. is monotone on the real reflexive B-space X.
(H2) The functional cp: X -+ ] -oc, 00] is convex, lower semicontinuous, and
tp f= +oc.
(H3) One of the following three conditions is satisfied:
(i) A: X --. X. is single-valued and hemicontiouous.
(ii) A is maximal monotone and int D(A)" D(Gcp) #: 0.
(iii) A is maximal monotone and D(A) n int D(ocp) #: 0.
(H4) The sum A + ctp: X .-. 2 x . is coercive with respect to 0, i.e., there are an
r > 0 and a Uo E D(A) '"' D(otp) such that
(u.,u - "0) > 0 for all (u,u.) E A + ccp with lIuli > r.
Proposition 32.36. Assllnae (H I) through (H4). Then. the original variational
inequalit}' (103) has a solution.
PROOF. By Proposition 32.17, the subgradient cqJ: X -+ 2 x . is maximal
monotone.
Ad(i). This is a special case of (ii).
Ad(ii), (iii). According to Theorem 32.l t the sum A + ct[): X .-. 2 x . is maxi-
mal monotone. Hence it follows from (H4) and Corollary 32.27 that the
equation (104) has a solution. This equation is equivalent to (103). 0
32.17. Application to Evolution
Variational Inequalities
We want to solve the variational inequality
(Lu + Au, v - u)  <p(u) - q>(v) for all v E X, (105)
where we seek u e D(L) " D(A} n D(ocp). By definition of the subgradient ocp
in Section 32.3c, problem (105) is equivalent to the equation
o E Lr4 + Au + ocp(u}, U E X. (106)
We assume:
(H 1) The linear operator L: D(L} s; X -+ X. is maximal monotone on the real
renexive B-space X.
(H2) The mapping A: X -. 2 x . is monotone.
(H3) The functional qJ: X -+ ] -00, 00] is convex, lower scmicontinuous, and
tp  +oc.
(H4) One of the following three conditions is satisfied:
(i) A: X -+ X. is single-valued and hemicontinuous.
(ii) A is maximal monotone and iot D(A) r\ D(ocp) #: 0.
(iii) A is maximal monotone and D(A}" int D(otp) #: 0.

894
32. Maximal Monotone Mappings
(H5) The sum L + A + acp: X -+ 2 x . is coercive \\'ith respect to 0, i.e., there
arc an r > 0 and a Uo e D(L) " D(A) " D(atp) such that
(u., u - uo) > 0 for all (u, u*) E L + A + all' with lIuli > r.
(H6) D(L) n int(A + oq»  0.
Theorem 32.J. Assume (H I) through (H6). Tllen the original var;atio,UJI in-
equality (105) has a solution.
PROOF. As in the proof of Proposition 32.36, we obtain that A + oqJ: X -+ 2 x .
is maximal monotone. It follows from (H6) and Theorem 32.1 that L +
(A + oq»: X  2 x . is maximal monotone. By (HS) and Corollary 32.27, prob-
lem (106) has a solution. This problem is equivalent to (105). 0
EXAtttPLE 32.37. (Evolution Variational Inequalities). We consider the special
casc, where X = L,(O, T; V), I < p < 00, and either
Lu = u', D(L) = {u E W,I(O, T; V. H): u(O) = O},
or
Lu = u',
D(L) = {u E W,l(O, T; V, H): u(O) = u(T)}.
Here, "V c H c V." is an evolution triple and 0 < T < 00.
Then, by Proposition 32.10, assumption (H I) above is fulfilled. In this case,
the original problem (105) is called an evolution variational inequality.
32.18. The Regularization Method for Nonuniquely
Solvable Operator Equations
We want to solve the equation
bEAu,
u eX,
( 107)
by means of the family of regularized equations
bEAu" + £.. Bu lt ,
u.. eX,
n = 1, 2, . . . ,
( 108)
where
t.. > 0
for all n
and
t. -+ 0
as n -+ 00.
( 109)
For given fixed b e X., let .</ denote the set of all the solutions u of (107). Our
goal is to show that
U" -t U
as n -t 00,
where u solves the original equation (107) and, at the same time, u is the unique
solution of the variational inequality
(Bu,v - u) > 0 for all ve!/ and fixed u e .9'. (110)

32..18. The Regularization Method (or Nonuniquely So1vable Operator Equations 895
Notice that we do not assume that the original equation (107) possesses a
unique solution. However, the regularized equation (108) is uniquely solvable
for each n. We make the following assumptions:
(1-11) The mapping A: X .... 2 x . is maximal monotone on the real reflexive
B-space X (e.g., A: X -. X* is monotone and hemicontinuous).
(H2) A is coercive with respect to the given fixed b e X., i.e., there is an r > 0
such that 0 e D(A) and
(u. - b,u) > 0 Cor all (u,u*)e A with 11111: > r.
(H3) The operator B: X  X. is strictly monotone, hemicontinuous, and
bounded. Moreover, 8(0) = o.
(H4) The given sequence (t,.) of real numbers satisfies (109).
Theorem 32.K (Convergence of the Method of Regulari7.ation). Assume (H 1)
through (H4). LeI b e X. be given. Then:
(a) The solutioll set .9' of ti,e origillal problem (107) is not empt}t, convex, and
closed in X.
(b) For each '1 e N, the regularized equation (108) has a unique solution u".
(c) As II  00, the sequence (u,,) converges \veakl)7 in X to a solution u of the
original equatiolJ (107), where u ;s the unique solution of the variational
inequalit)7 (110).
(d) If the operator 8 satisfies cOlJdition (S tllen (un) cO'lverges strongl)' in X
10 II.
PROOF. For simplicity of notation, the elements u* of Au arc sometimes
denoted briefly by Au.
Ad(a). By Corollary 32.27, the solution set -.f/ of (107) is not empty. Since
A is maximal monotone, we have u e f/' iff
(b - v*, u - v)  0
for all (I', v.) e A.
Hence Y is convex and closed in X.
Ad(b). The operator B: X -+ X. is maximal monotone. According to
Theorem 32.1, the sum A + £,,8: X  2 x . is maximal monotone. Since 8(0) =
O. the monotonicity of B implies
(8u,u)  o.
From (H2) we obtain
(rl. + £,,8u - b,u) > 0
for all (u, u*) e A with !lu II > r.
Hence 0 E D(A + t"B) and
(v* - b, u) > 0 for all (u, v.) e A + £"B with )lull> r.
By Corollary 32.27, the regularized equation (108) has a solution u" for each n.
To prove the uniqueness of rl", we suppose that U II and v" solve (108) for

896
32. Maximal Monotone Mappings
fixed n, i.e.,
Au" + £"Bu" = b
and
Av" + e"Bv" = b.
Hence
o = (b - b, II" - v,,) = (Au" - Av", U" - v,,) + £,,(Bu. - Bv", u. - VII)
> t,,(Bu II - Bv", U" - v,,),
by the monotonicity of A. Since B is strictly monotone. u. = V".
Ad(c).
(I) We prove first that the variational inequality (110) has at most one
solution. Indcc<t let u and ", be solutions of (110). Setting v = '" and
v = 14, we obtain
(Bu - BM', u - \v) < o.
The strict monotonicity of B implies u = "'.
(II) A priori estimates. We want to show that
lIuall s: r
for all n
and all solutions 14" of( 108). Otherwise, there is a solution II" oft 108) with
lIu,,11 > r. From (108) and the coerciveness condition (H2), we obtain
(Au" - b, u ll ) = ( -t.Bu", u,,) > o.
On the other hand, it follows from B(O) = 0 and from the monotonicity
of B that (Bu", u,,)  o. This is a contradiction.
(III) Since (14,,) is bounded, there exists a sequence, again denoted by (II,,), such
that
I' ll  II
as n -+ 00.
We want to show that II e //. Using the monotonicity of A, we obtain,
for all (v, v*) E A,
o  lim (Au. - v.,u" - v)
"-Q)
= lim (b - £"BII" - v.,u" - v)
" ... 
=(b-v.,u-v).
In this connection, note that (Bu,,) is bounded, since B is bounded.
Because of the maximal monotonicity of A, we get bEAu, i.e., u e /1'.
(IV) We want to prove that u is a solution of the variational inequality (110).
To this end, we set
u, = u + leV - u)
for 0 < l S 1 and v E .9:
where t and v are fixed. Since 9' is convex. we obtain u, e  and hence
beAu,. From the regularized equation (108) it follows that
o = (Au" - All" 14" - u,) + £"(Bu,,, UII - u,)
 £.(Bu", U" - II,)
for all n.

32.19 Characterization of linear Maximal Monotone Operators
897
Hence
o > <Bu", u" - u,) = (Bu" - Bu,. u" - u,) + (Bu" u" - u,)
> (Bu" u" - u,).
Letting n -+ x, we get
o > (Bu" u - u,) = t<Bu" u - v).
Dividing by t and letting t -+ + 0, we obtain
(Bu,l'-u» O
for all v E //,
i.e., u is a solution of (110).
(V) According to our considerations above, each subsequence of (u,,) has, in
turn, a subsequence which converges weakly to the unique solution u of
(110). By the convergence principle (Proposition 21.23{i)), the entire
sequence (u,,) converges weakly to u.
Ad(d). From (108) and bEAu, we obtain
o = (Au" - b + f."Bu", u" - u) > £"(Bu,,, u" - u)
and hence
o > (Bu", u" - u) = (Bu" - Bu, u" - u) + (Bu, u" - u).
Noting that 14"  U as n -+ oc, this implies
o  (Bu" - Bu, u" - u) -+ 0 as n -+ oc.
Since B satisfies condition (8), we obtain that u" -+ u as n -+ oc.
D
32.19. Characterization of Linear Maximal
Monotone Operators
Theorem 32.L (Brezis (1970)). f"or a linear operator L: D(L) c X -+ X. on the
real reflexive B-space X, the following t'o statements are equil'alent:
(i) L;s maxin1al monotone.
(ii) D( L) is dense in X t Land L. are monotone, and L ;s graph closed.
PROOF. To prove that (ii) implies (i), we use a minimum problem and the
duality maps on X and X..
( i) => (i i ).
(I) D(L) is dense in X. Otherwise, it follows from the Hahn Banach theorem
that there exists a functional hEX. such that h  0 and
(b,u) = 0
for all u E D(L).
Hence
(Lu - b, u) = (Lu, u) > 0
for all u E D(L).

898
32. Maximal Monotone Mappings
Since L is maximal monotone, this implies h = L(O) = 0, which is a
contradiction.
(II) L is graph closed. To prove this, let
14,. -+ U
and
Lu,. -+ b
as n -+ 00.
From
(Lu,. - Lv, U,. - v) > 0
we obtain
for all v E D(L) and all '1,
(b - Lv, u - v) > 0
for all v E D(L)
and hence b = Lu.
(II I) L * is monotone. Since L * is linear, it is sufficient to prove that
(L*u,u) > 0
First, let u E D(L) fl D(IJ .). Then
(L *u, u) = (u, Lu) > o.
for all U E D(L *).
Secondly, let u E D(L *) and u f D(L). Since L is maximal monotone,
there exists a v E D(L) such that
(Lv - h, l' - u) < 0,
where h = - L .u. Hence
(Lv,v) < (Lv,u) - (L*u,v) + (L*u,u) = (L*u,u).
Since (Lv, v) > 0, we obtain (L *u, u) > o.
(ii) => (i). Passing to an equivalent norm on X, we may assume that X and
X. are strictly convex. We have to show that
(Lu - b, u - v) > 0
for all U E D(L)
(III)
implies b = Lv. To prove this, we consider the minimum problem
g(u, u.) = min !,
where (v, b) E X x X. is fixed and
g(u, u*) = (u* - b, u - v) + IIu. - blli. + IIu - vll.
(u, u.) E G(L),
( 112)
By (ii), L is graph closed, i.e., G(L) is a closed linear subspace of X x X*. The
functional g: G(L) -+ R is convex and continuous. From the well-known in-
equality + ab  !a 2 + !b 2 , we obtain
g(u, u*) > ! lIu. - bll 2 + ! lIu - vII 2,
and hence g(u, u*) -+ +00 as Ilull + IIu*1I -+ 00. According to Theorem 25.E,
the minimum problem (112) has a solution (u, u*) E G(L), which satisfies the
Euler equation
g'(u,u*)(IJ,h*) = 0
for all (h, h*) E G(L).

32.20. Extension of Monotone Mappings
899
Explicitly, that means
(h.,u - t') + (u. - b,h) + 2J(u. - b)h. + 2J(u - v)h = 0, (113)
for all (h, Iz.) E G(L), where J: X  X. and J: X.  X denotes the duality map
on X and X., respectively. The condition (u,u.), (h,h.)e G(L) means that
II. = Lu and h. = Lh. To simplify notation, we set
x=u-t'
and
y = Lu - b.
By ( I I 3),
(Liz, x + 2Jy) = - (2Jx + y. h) for all h E D(L).
Hence L*(x + 2Jy) = -(2Jx + y). This implies
- (2J.'( + y, x + 2Jy) > 0,
since L. is monotone. By ( III), (y, x> > O. Hence
- -
(J):,.\:) + (y,Jy) + 2(Jx,Jy)  O.
Noting that (Jx,x) = IIxl1 2 and <Jx,Jy) > -IIJxIlIlJYII = -lIxlillyll, we
obtain
II X 11 2 + "J''' 2 - 2" x 1111 }' II < O
and hence x = }' = 0, i.e., u = v and Lv = b.
D
32.20. Extension of Monotone Mappings
Proposition 32.38. Let C be a nonempty closed conl'ex subset of the real rej7e.xive
B-space X, and let t he mapping
A: C --+ 2 x .
be maximal monotone. If K'e set
- { Au
Au =
o
if U E C,
if U E X - c,
- .
then A: X ..... 2 x is also maximal monotone.
PROOF. Passing to an equivalent norm on X, we may assume that both X and
X. are strictly monotone. By Proposition 32.22, the duality map J: X -+ X.
is single-valued, monotone, bounded, and demicontinous, and hence J is
pseudomonotone. For each Uo EX, .
. <Ju,u - u o ) .
hm I - > hm lIuli - lIuoll = +OC'.
II..II-XJ lJul .."-r
By Corollary 32.25 R(A + J) = X..

900
32. Maximal Monotone Mappings
- - .
Hence R(A + J) = X*. Thus, by Theorem 32.F, the mapping A: X -+ 2 x
is maximal monotone. 0
The following theorem shows that each monotone mapping can be extended
to a maximal monotone mapping.
Theorem 32.M (Extension Theorem). Let
A: X ..... 2 x .
be a mOllotolJe mapping on the real reflexive B-space X with D(A) :1= 0, i.e., A
is not the empty map. Then there e.x;sts a maximal monotone mapping
- .
A: X -+ 2 x
-- -
such that G(A) c G(A) and D(A) c co D(A).
PR()()F. We use Zorn's lemma. To this end, set C = co D(A). Denote by !/ the
set of all monotone mappings B: C -+ 2 x . such that 8 is an extension of A.
For B 1 , 8 2 E .'i, we write
BJ < B 2 iff G(B 1 ) C G(B 2 ).
This way, ,l/ becomes an ordered set. Let ft be a chain, i.e.,  is nonempty
and B., 8 2 E r& implies B 1 < B 2 or B 2 < B.. If we set
Su = U Bu
Bc.
for all u E C,
then the mapping S: C -+ 2 x . is monotone and
B < S
for all B E fG,
i.e., S is a upper bound for the chain fie
By Zorn's lemma, there is a maximal element Ao in C/, i.e., Ao  Band
B E .l/ implies B = Ao (cf. Problem 11.1 b).
Obviously, the mapping Ao: C  2 x . is maximal monotone. We set Au =
Aou if u E C, and Au = 0 if u E X-c. By Proposition 32.38, the mapping
A: X --+ 2 x . is maximal monotone. From A  Ao, we obtain
-
G(A) c G(Ao) = G(A}
and
-
D(A) = D(A o ) c C.
o
EXAMPLE 32.39. Let X = R. We consider the monotone mapping
A: R  2 R
with D(A) = ]a, b[ as pictured in Figure 32.8(a). Then the mapping
A: IR  2 R ,
pictured in Figure 328(b), is the unique maximal monotone extension of A
with the property D(A) = co D(A) = [a, b].

32.21. J-Monotone Mappings and Their Generalizations
901
A
-
/A
a
b
a
b
(a) monotone mapping
(b) maximal monotone extension
Figure 32.8
32.21. 3-Monotone Mappings and
Their Generalizations
Let us recall that, by definition, a mapping A: X --+ 2 x . on the real B-space X
is cYf!ic monotone iff the condition
(uT,u 1 - U2) + (u!,u 2 - u 3 ) + ... + (u:,u n - u n + 1 ) > 0 (114)
holds for all (u;, u) E A, i = I, ... t n, and all n = 2, 3, ..., where we set
U n + 1 = U10 In particular, the single-valued mapping A: D(A) £; X --+ X. is
cyclic monotone iff
< A Us' U I - U 2) + < Au 2' U 2 - U 3) + ... + (A Un' Un - Un + I ) > 0 ( ) 14.)
holds for all U i E D(A), i = 1,..., n, and all n = 2,3,..., where we set U"+I = Us.
Definition 32.40. Let A: X --+ 2 x . be a mapping on the real B-space X.
(a) A is called n-monOlone iff condition (114) holds for fixed n > 2. In
particular, A is 3-monotone iff
(v. - u., \\' - v)  (u. - w., u - w)
holds for all (u, u.), (t" v.), (w, w.) E A.
(b) A is called 3-l1-monotone iff A is monotone and there is a number l1 > 0
such that
«(7. - u., W - v) S; l1(u. - w., U - ",.)
holds for all (u, u.), (v, v.), (w, w.) E A.
(c) A is called 3.-monotone iff A is monotone and
sup (v. - u., w - v) < 00
(I.. t'.) E A
holds for all 'E D(A), u. E R(A).

902
32. Maximal Monotone Mappings
monotone
Nemyckii
operator
 subgradient acp

cyclic monotone
t
n-monotone
for each n

3-monotone

. 3-a-monotone

monotone with
bounded range
linear, monotone,
and symmetric
I " 
Inear, monotone,
and angle-bounded
*
linear and
3-a-monotone
monotone and
st rongl y coerci ve
.
uniformly monotone
 
llammerstein
integral equations
monotone mapping
quasi-linear
elliptic
differential
equations
f;igure 32.9
Figure 32.9 shows important interrelations which will be proved below. The
notion of 3.-monotonicity will playa decisive role in the next section.
Proposition 32.41 (3*-monotonicity). Let A: X -+ 2 x . be a mapping on the real
rejlexive B-space X.
(a) If A is n-monotone, then A is monotone.
(b) If A ;s 3-monotone, then A is 3-a-monololle with a = I.
(c) If A is 3-a-monotone, then A is 3..monotone.
(d) If A ;s monotone and stro1Jgly coercive (e.g., A: X --+ X. is uniformly
monotone), then A is 3.-monotone.
(e) I}O A is monotone and the range R(A) ;s bounded, then A is 3.-monotone. If,
in addition, A is maximal monotone, then D(A) = X.
(f) If A ;s 3.-monotone, then so ;s A -1: X. --+ 2 X .
By definition, the mapping A: X -+ 2 x . on the real B-space X is strongly
coercive iff either D(A) is bounded or D(A) is unbounded and
< v. , v - w)
-+ +00
II vII
as II vII -+ 00, (v, I).) E A,
( 115)
for each \\' E D(A). More precisely, this means that, for each given R > 0 and

32.21. 3.Monotonc Mappinp and Their Generalizations
903
'" e D(A), there exists an r(Mo') > 0 such that
(v*. v - w) > R for all (v, v*) e A with IIvll  r(w).
II vII -
For example, suppose that the operator A: X -. X. is uniformly monotone,
i.e.,
(Av - A\v, V - \v) > a(ilv - \v11)lIv - "'[1
for all v, '" E X,
\\'here the continuous function a: R. -+ R is strictly monotone with a(O) = 0
and a(t) -+ +:x> as t -+ +00. Then A is strongly coercive. This follo\\'5 from
( A v, v - \,,)  a ( II v - '" 11) II v - w II - II A \v lilt v - 'v II.
PROOF. Ad(a). (b (c), (f). This is an immediate consequence of the corresponding
definitions.
Ad(d). Let ,,,. e D(A) and u* e R(A). We choose a fixed \v. E A\". It follows
from (115) that there is an r > 0 such that
11 c!!.f' (v* - u*, w - v)  O.
for all (v. 1'*) e A with IIvll > r. If Ii vU < r, then the monotonicity of A implies
(v. - u*, ", - v)  <Mo'* - u., w - v) for all (v, v.) e A,
and hence
11  (11\,,*1} + II u* 11)(11 wll + r),
for all (v, v*) e A with Jlvll < r. Thus SUP(lt.I'.) it A < 00.
Ad(e). Let \\-' E D(A) and 11* e R(A). We choose 'v. and u such that ("'. ",'*),
(U,II*) e A. From the monotonicity of A it follows that
(v. - u*,w - v)  (v* - u.,\" - v) + (v. - u.,v - u)
= (v. - u., \v - u) for all (v, v*) E A.
Since R(A) is bounded, the mapping A is 3.-monotone.
If A is maximal monotone, then so is A -I. Since R(A) is bounded, the
mapping A is bounded. By Theorem 32.G the mapping A -1 is surjective i.e.
D(A) = X. 0
Proposition 32.42. Let q>: X -+ ] -oc, oc] be a functional on tile real B-space
x. TheIl the subdifferenlial
alp: x ... 2".
is c)7clic monotone, i.e., alp is n-monolone for each n > 2.
IPJ particular, alp is 3*-monotone.
PROOF. If (u h u?) E iJq> for i = 1,..., n, then
(ur, Ui"l - Ui)  lp(U i + 1 ) - CP(Ui
i = I,..., n,
where U n + 1 = u 1 . Addition shows that ocp is n-monotone.
o

904
32. Maximal Monotone tappjngs
Proposition 32.43. Let K: X  X. be a linear monotone operator on tile real
B-space X. Then tile follo\\';ng '\"0 conditions are equivalent:
(i) K;s angle-bounded, i.e., there is a nunJber a > 0 such that
I(KU,t,) - (Kv,u)1 2  4a 2 (Ku,u) (Kv,v)
for all u,veX. (116)
(ii) K is 3-t1-monotone, i.e., there ;s a number t1 > 0 such tl,at
(Kv - KII, \\' - v) S t1(Ku - Kw, u - \\') for all u, v, ", e X. (117)
PROOF. We first show that (117) is equivalent to the inequality
(Ku, V)2 :s; 4a(Ku, u) (Kv, v) for all u, veX. (118)
In fact, letting x = u - '''' and y = v - \\. and noting the linearity of K, we
obtain that (117) is equivalent to
(Kx,y) - (Ky.}') S t1(Kx,x)
for all .:t, ye X.
( liS.)
Replacing x by tx, \VC get
a(K.,,=,x),2 - (Kx,).)t + (K)"y)  0
Consequently, (118*) is equivalent to (118).
We now set
for all , E IR.
[v] i = !( (Ku, v) 1: (Kv, u»
for all u, veX.
Since K is monotone, we have [u, u].  0 for all u e X. By the generalized
Schwarz inequality (Problem 21.16),
[u. v] S [u, u]+ [v, v]... for all II, veX.
Obviously,
(Ku,v) = [utv]+ + [u..v]- rorall u,veX. (119)
(I) If K is angle-bounded, then
[II, v]: sa 2 [u,u]+[v,v]. forall u,veX.
By (119) and (Cf + fJ)2 < 2(X2 + 2{J2 for real a, p, we obtain
(KU,V)2 S (2 + 2a Z )[u,u].[v,v). for all u, VEX.
This is (118), i.e., K is 3-a-monotone.
(II) Conversely, if K is 3-a-monotone, then (118) holds. From (119) we obtain
[II,V]_ = (KII,V) - [u,v].
and hence
[II,V]  (8a + 2)[u,u];[v,v]
This is (116 i.e., K is angle-bounded.
for all II, veX.
o

32.21. 3-Monotone Mappings and Their Generalilations
905
J."inally, we consider the Nem}.cki; operator f: X -. X.  where
(Fu)(x) = f(x, u(x))
for all x e G
and X = Lp(G). OUf assumptions are the following:
(HI) Let G be a nonempty bounded measurdble set in R'v, N  I, and let
I < p, q < 00 with p-l + q-I = I.
(H2) MonotonicilY. The function f: G x IR..... R satisfies the Caratheodory
condition (e.g., f is continuous), and u....... f(., u) is monotone increasing
on IR for almost all . e G.
(H3) Gro\vtlJ condition. There is a function a E L 4I (G) and a number b > 0 such
that
If(x, u)1 S la(x)1 + b lul P - 1
for all x e G. u e R.
Proposition 32.44. Assume (H 1) through (H3). ThelJ the Nem}.ckii operator
F: X -. X. is cyclic monotone, i.e., F is n-monotone for each n  2.
In particular, F is 3*-monotone.
In Proposition 41.10 we will prove the stronger result that F: X ..... X. is a
continuous potential operator.
PROOF. We set
g(x, v) = f' f(x, u) dr,
and
h(u) = fG g(x, u(x» dx.
By (H2). the function v g(x. v) is convex and CIon R for almost all x e G.
This implies
g(.. v) - g(., ",) > (v - w)g,,(x. \,,) = (v - \v)f(x. "'),
for all v, w e R and almost all x E G. By Proposition 26.7, the operator
F: X ..... X. is continuous. Recall that X = L,(G) and X. = Lq(G). Since
Ig(x, v)1  la(x)11 vi + bp-ll viP for all x E G, v e R,
the integral !oy(x.t'(x)dx exists for all veX, by the Holder inequality.
Therefore, integration yields
h(v) - h(w) > (F,,', v - ",)
for all v, \v e X.
Letting
w = u.
.'
v = Ui. 1 ,
; = 1, . . ., II, u.+ 1 = U 1 ,
and adding the corresponding inequalities, we obtain that F is n-monotone
for each IJ. 0

906
32. Maximal Monotone Mapping.
32.22. The Range of Sum Operators
Our goal is to prove that
R(A + B)  R(A) + R(B),
( 120)
i.e.. the range R(A + B) of the sum operator A + B is '.almost equal" to
R(A) + R(B). More precisely, we will use the symbol "" in the following
sense.
Definition 32.45. Let Sand T be subsets of a topological space X (e.g., X is a
B-space). We write
ST
iff
int S = int T
and
- -
S = T.
In this case, we say that the set S is almost equal to the set T.
Recall that iot Sand S denotes the interior and the closure of the set S,
respectively.
For example, if T is a B-space, then S  T means S = T. We now want to
study the equation
bEAu + Bu,
UE X.
(121)
Theorem 32.N (Brezis and Haraux (1976». Assume:
(i) The operators A, B: X -+ 2 x are maximal monotone and 3*-monolone on
ti,e real H-space X.
(ii) Tile sunl operator A + B: X -. 2 x is maximal mOllotone (e.g., D(A) r\
int D(B)  0).
-[lien
R(A + B)  R(A) + R(B).
In particular, if R(A) = X or R(B) = X, then R(A + B) = X, i.e., equation (121)
lIas a soilltion for each gitn b eX.
Corollary 31.46. The assertion remains t",e if we replace the 3.-monotonicity
of the operator A by tile condition D(A) C D(B).
The proof will be based on the following crucial result.
Lemma 32.47. Let C: X -+ 2 x be a maximal monotone operator on the real
H-space X. Let S be a subset of X sucll that, for each u* e S, there exists a
\\. E X ,vith
sup (v. - u*I\" - v) < 00.
(.p.)C
( 122)
TheIl, coS  R(C) and int(coS) s; R(C).

32.22 The Range of Sum Operators
907
PROOF OF LEMMA 32.47. We will use critically the uniform boundedness
principle of Banach and Stein haus.
(I) We show that S s R(C). Let u. e S. Since C is maximal monotone, the
equation
Cv£ + £V r = u.,
VI e D(C)
( 123)
has a solution for each t > O. In this connection, note that C is maximal
accretive by Proposition 31.5. By assumption (122), there are a '" e X
and a real number c such that
(v. - Il*lw - v)  c
for all (v, v*) e C.
We set v = v, and v. = u. - tv,. Hence
(£vclv t - w)  c
for all £ > O.
This implies
E: II v t: 11 2 S £ II v (II II w II + c  ! l: 211 V r 11 2 + ! II \V 11 2 + c.
Thus the sequence (jt IIvelD is bounded as t - O. By equation (t 23).
CV t -. u.
as £ -.0, i.e., Il. e R(C).
(II) We show that int S s R(C). Let u* e int S. We choose r > 0 such that
u. + \v. e S for all \v. with II w*1I < r. By assumption (122), for each
".* e X with II '''*11 < r, there are a \v e X and a real number c such that
(v. - u. - "'.1 w - v)  c
We set v = VI: and v* = u* - €v&. Hence
(tv, + "'.Iv t - w) < c for all I; > O.
By (I), the sequence (t II veil 2 ) is bounded as € ..... O. Therefore. the sequence
{ (\v.1 v,)} is bounded as I: -. 0 for each w. e X with 11"'*11 < r. By the
uniform bOllndedness theorem AI (35), the sequence (jJvlI) is bounded as
I; -. O. Consequently, there exists a subsequence with
for all (v, v*) e C.
V,  v as £ -. O.
From equation (123) it follows that u. - We E CV e for all t > 0 and
u* - tv  u*
,
as I: -. O.
Since C is maximal monotone, we obtain that u. e Cv, by Proposition
31.6. Thus u. e R(C).
(III) We show that the assumption (122) is also satisfied for the convex hull
co S. In fact, if u. e co S, then
u. =  l,Ur,
L 'i = I,
I
where ur e Sand '.  0 for all i. By assumption (122), there are elements

908
32. Maximal Monotone Mappings
Wi and numbers C i such that
(v. - utlw; - 1') < C..
for all (v, v.) E C and all ;.
Letting w = Li t,w i and noting that Li t i = I, we obtain
(v. - u.lw - v) < L tiC. + L 'i(Urlw.) - (u.lw),
I i
for all (v, l'.) e C. This is (122) for co S.
(IV) According to (III), we can replace the set S by co S in (I) and (II). This
yields the assertion. 0
PR()()F OF THEOREM 32.N. Obviously,
R(A + B) c R(A) + R(B).
Hence, it is sufficient to show that
R(A) + R(B) c R(A + B)
and
int(R(A) + R(B)) c R(A + B).
To prove this, we want to apply Lemma 32.47 to the operator
C=A+B
and to the set S = R(A) + R(B). Thus it remains to check the assumption (122).
Let u. E S, i.e., u. = u: + u; with u: e R(A) and u; E R(B). Choose a fixed
w E D(A) n D(B). Note that D(A)" D(B) :F 0, since A + B is maximal mono-
tone and hence A + B =F- 0. Since A and Bare 3..monotone, we have
sup (v. - u:lw - (J) < 00,
h'.V.)E A
( 1 24a)
sup (v. - u:lw - v) < 00.
(V.V.)E B
( 124b)
This implies
sup (v. - u.lw - v) < 00,
(V.V.)EC
( 125)
i.e., condition (122) is satisfied.
o
PR()()FOF COROLLARY 32.46. In contrast to the preceding proof, we now choose
w such that w E D(A) and u; E Aw. Since D(A) £ D(B), we again have
W E D(A) n D(B). From the monotonicity of A it follows that
(v. - u:lw - v) < 0 for all (v, v.) E A.
This is (124a). From (124a) and (124b) we again obtain (125).
o
32.23. Application to Hammerstein Equations
We consider the abstract Hammerstein equation
b E K fu + u,
U E X.
( I 26)

32.24. The Characterization of Nonexpansi\'e Semigroups in H-spaces
909
Theorem 32.0. Suppose lhallhe IJUlpp;ngs K, I:: X ... 2. t are max;nUlI mOnOIOIIe
011 the real H-space X ,v;th D(K) = D(F) = X, and at least one of these ""0
mappings is 3.-I1Jonotone.
Tllen. for each be X, equation (126) has a solution.
For example. the assumptions of Theorem 32.0 are satisfied if the following
conditions hold:
(i) The operator K: X -+ X is linear and monotone.
(ii) The operator F: X  X is continuous and monotone.
(iii) The operator K is angle-bounded or F is a potential operator or F is
strongly coercive or the range of F is bounded.
In contrast to Theorem 32.8, the theorem above also applics to operators
I: which are not coercive.
PROOF. We apply Corollary 32.46.
(I) Suppose that K is 3*-monotone. We consider the equation
bEAt' + Bv, VEX. (126*)
,,'ith A = F- 1 and B = K. We have R(A) = D(F) = X and D(B) = X. By
Corollary 32.46, R(A + B) = X, i.e., (126*) has a solution v for each b EX.
Choosing '4 E Av, we obtain a solution u of the original equation (126).
(II) Supposc that F is 3*-monotonc. Thcn equation (126) is equivalent to
o E Au + Bu. u EX.
\\'here Au = -K- 1 (b - u) and Bu = Fu. Since K is maximal mono-
tone. so is A. Moreover, we have R(A) = D(K) = X and D(B) = X. By
Corollary 32.46, R(A + B) = X. 0
An application of this theorem to Hammerstein integral equations in L 2 (G)
has already been formulated in Proposition 28.4. In this connection, observe
Proposition 32.44 on the Nemyckii operator.
32.24. The Characterization of Nonexpansive
Semigroups in H -spaces
We consider the following multivalued initial valuc problem
u' + Au 3 0,
u(O) = U O '
( 127)
We assume:
(H) The operator A: X ..... 2 x is maximal monotone on the real H-space X.

910
32. Maximal Monotone Mappings
Definitioa 31.48. Let Uo E D(A). By a generalized solution of (127 we under-
stand a Lipschitz continuous function
14: [0, oo[ -+ D(A)
such that u(O) = U o and
u'(t) + Au(t) 3 0
for almost all t e ]0, 00[,
where the derivative u'(t) is to be understood in the classical sense (cf.
Definition 3.4).
If u = lI(r) is a generalized solution of (127), then we set
U(I) = S(t)u o for all t > o.
Under the assumption (H the set Au is noncmpty, closed, and convex for
each u e D(A), by Proposition 32.6. Thus, the minimum problem
min IIvll = IIvoll
"e Au
has a unique solution vo, by Theorem 2S.E. We set
Aou = Vo.
This way we obtain the single-valued operator Ao: D(A) S X -+ X (Fig. 32.10).
The following theorem shows that, on real H-spaces, there exists a one-to-
one correspondence between maximal monotone operators and nonexpansive
.
semlgfOups.
Theorem 32.P (Crandall and Pazy (1969». Let X be a real H-space.
(a) If the mapping A: X -+ 2% is maximal monotone. then tile operator - Ao is
the g enerator of a un iq ue I)' determined nonexpansive semigroup {S(t)} on
DCA).
J'tIore precisely, for each Uo e D(A), the initial value problem (127) has a
unique generalized solution u = u(t), and there exists a unique Iwnexpans;ve
./A
Ao
(a)
(b)
Figure 32.10

Problems
911
semigroup {S(t)} on D(A) such that
U(l) = 5(t)uo for all t > o.
(b) COIJverselY9 if {S(t)} ;s a nonexpans;ve sem;group on the nonempty closed
convex .-.ubsel C of x then there exists a uniqu ely determined maximal
monotone mapping A: X ..... 2% such that C = D(A) and - Ao is tile genera-
tor of {S(t)}.
More precisel:}' the semigroup {S(t)} can be constructed as in (8).
This theorem generalizes the main theorem on linear nonexpansive semi-
groups (Theorem 19.E). Moreover, this theorem generalizes Theorem 31.A
to multivalued mappings. The proof of Theorem 32.P will be discussed in
Problem 32.8.
PROBLEMS
32.1. Characterizat;oIJ of the weak closure by means of sequences. Let J\1 be a
bounded subset of the reOexivc B-space X, and let u be a point in the weak
closure of M. By A J (17c), there exists an M -S-scquence (u.) in .\1 such that
u-..u in X.
Prove that there exists a sequence (u.)".,.. in M such that
u.u in X
as n -. 00.
( 128)
This result shows that:
"'eak closure of JW = sequentially weak closure of M.
Hint: Use the following general results.
(i) The weak topolog)' on a weakly compact subset of a separable B-space is
a metric topology.
(ii) The ",'cak topology on a bounded closed convex subset of a renexive
separable B-spacc is a metric topology.
The proof of (i) can be found in Dunford and Schwartz (1958, M). Vol. 1.
p. 434. According to the Eberlein-Smuljan theorem. statement (ii) is a special
case of (i).
Solution:
(I) Since X is renexive, so is X.. Moreover, each finite product X* x'.. x
X. is also a reflexive B-space. FinallYt each closed linear subspacc Xo of
X is reflexive.
(II) Let u be fixed. We prove first that there exists an at most countable subset
J\1 o of JW such that u lies in the weak closure of Mo with respect to the
weak topology on X. Let B" be tbe product of n copies of the closed unit
ball Bin X*. Choose fixed natural numbers m and n. For (/1 t... .f,.) E B"t
we set
U(u) = {VE X: I(Ij.v - u)1 <  ,j = l,....n}.
Since u lies in the weak closure of M. there exists a v E M such that

912
32. Maximal ionotonc Mappings
Ii e U (u), i.e.,
1
I(jj. v - u)1 < -,
m
For fixed Ii e M, the set
V = {<fl.... ./.) E 8": inequality (129) holds}
represents a \\'cakly open subset of 8". By the Eberlein - Smuljan theorem.
B is weakly compact in the reflexive B-space X.. Moreover. 8" is \\'cakly
compact in the reflexive B-space (X.r. All the \\'cakly open sets V cover
8 n . Since B" is weakly compact, 8" can be covered by finitel)' many sets
of t)'pe  This means that there exists a finite subset S.. of At such that,
for each (fl' . · . ,f,.) e 8", there exists a v E Snrtl satisfying (129).
We now set
j := I,..... n.
(129)
,W o = US....
....e"
Then. for each n, meN and each (I.,. II ..In) e 8 n , thcre exists a v E j\e1o
such that (129) holds. i.e.. the point U lies in the \\'eak closure of J'lo with
respect to the weak topology on X.
(III) Let Xo denote the smallest closed linear subspacc of X which contains
Mo and u. Then Xo is a separable and reflexivc B-space by (I). According
to (II), the point u lies in the weak closure of '\"0 with respect to the \\'cak
topology on X. By the Hahn-Banach theorem. each runctional.rE X 6
can be extended to a functional Ie X*. Therefore. U also lics in the \\'cak
closure of J\"o with respect to the weak topology on Xo.
No\\', choose a closed ball K in Xo such that ,'do S; K. According to
(ii) above. the "'cak Xo-topology on K corresponds to a metric. Thus.
there exists a sequence (u.) in Mo such that
U n .-.. U in Xo
as II -+ 'X).
This implies the assertion (128), since X. c X 3.
32.2. Conlinuit,. properties o.f multittalued maps. We consider the map
A: C .-.2 f .
( 130)
where C and Yare topological spaces. Then A is called upper semicontinuous
at the point u iff. for each open neighborhood V of the set Au, there exists a
neighbhorhood U of the point u such that
A(U) S 
Properties of such maps have been studied in Section 9.2.
Let X and Y be B-spaccs o\'cr [e( = R. C, and Ict C c: X. Then the map A
in (130) is called dem;con,;nuous at u iff A is upper scmicontinuous at u from
the strong topology on C into the weak topology on 1':
The map A in (130) is called strongly continuous at u iff A is upper semi-
continuous at u from the weak topology on C into the strong topolog)' on ):
Let the set C be convex. The map A in (130) is called hemicontinuous at u
iff. for each v e C. the map
t...... A(tu + (1 - t)I')

Problems
913
is upper semicontinuous from the interval [0, I] into the space Y equipped
with the weak topology.
The map A in (130) is called upper semicontinuous (resp. demiconunuous,
strongly continuous, hemicontinuous) iff A has the corresponding property at
each point u in D(A).
The map A is called bounded ifTthe image A(M) is bounded for each bounded
subset M of D(A).
32.2a. llemicontinuity and demiconlinuity. Obviously, each demicontinuous map is
also hemicontinuous.
Conversely, let
A: X -+ 2 x .
(131)
be hemicontinuous and monotone on the real reflexive 8-space X. Let (' be
an open subset of D(A) and suppose that, for each u Ell, the set Au is convex
and weakly closed in X.. Show that A is demicontinuous on U.
Hint: Use a similar argument as in the proof of Proposition 26.4. Cf. Kluge
(1979, M), p. 32.
32.2b. General monolonicily Irick. Consider A and U as in Problem 32.2a. Show that
it follows from (u, u.) E U x X* and
(u* - v.,u - l>x > 0
for all (v. v*) E A
that (u, u.) E A.
This result generalizes the fundamental monotonicity trick (25.4).
Hint: Assume (u, u*) f A and use the separation theorem for convex sets in
Section 39.1. Cf. Kluge (1979. M), p. 32.
32.3. PseudOmOIJOIOne mullil'alued maps. Let C be a closed convex subset of the real
renexive B-space X. The map
A: C  2 x .
( 132)
is called pseudomonotone iff the following holds. Let (u", u:) E A for all n E ,
and suppose that u"  u as n -+ 00 such that
lim (u:.u,,-u) < O.
" .... r,
Then, for each v E C, there is a u such that (v. u:) E A and
(u. u - v) < lim (u:. u" - v).
" .... :x)
32.3a. Show that each strongly continuous map A: C -+ X. is pseudomonotone.
Solution: If u"  u as n -+ 00, then Au" --+ Au.
32.3b. Show that each continuous operator A: C -+ X. is pseudomonotone in the
case dim X < oc;.
Solution: This is a special case of Problem 32.3a, since weak convergence
and strong convergence coincide on X.
32.3c. Let A: C -+ 2 x . be monotone and hemicontinuous on the nonempty closed
convex subset C of the real reflexive 8-space X. In addition. suppose that, for
each u E C. the set Au is nonempty, convex. and closed in X.. Show that A is
pseudomonotone.

914
32. Maximal Monotone Mappinp
Hint: Use a similar argument as in the proof of Proposition 27.6. Cf. Kluge
(1979. M), p. 193.
32.3d. Additivity. Let the maps A. B: X -+ 2 x . be pseudomonotone on the real reflex-
ive B-space X. Show that the sum
A + B: X -+ 2 x .
is pseudomonotone.
Hint: Use a similar argument as in the proof of Proposition 27.6(e).
32.4.. Ge,aeralizarion of the main theorem on pseudomono!one perturbations of maxi-
mal monotone mappings (Theorem 32.A). We consider the multivalucd operator
equation
b e Au + Bu,
ue C,
( 133)
where. in contrast to Theorem 32.A, both the maps A and B are multivalued.
We assume:
(i) C is a nonempty closed convex subset of the real renexi\'e B-space X.
(ii) The map A: C -+ 2%. is maximal monotone, and B: C ..... 2 x . is pseudo-
monotone and bounded.
(iii) B is A-coerci\'e with respect to the fixed b e X., i.e., there is a Uo E C r.
D(A) and a number r > 0 such that
(u.,u - u o ) > (b,1l - uo).
for all (u, u.) E B with II U II > r.
(iv) For each u e C, the set Bu is a nonempty closed convex subset of X*.
(v) B is demicontinuous on simplices, i.e., for each finite subset F of C, the
map B: co F -+ 2 x . is demicontinuous.
Show that problem (133) has a solution.
Hint: Passing to an cqui\'alent norm on X, if necessary, we may assume that
both X and X* arc strictly convex.
(I) Regularization. Instead of (133), we start with the regularized equation
b e (Au + £Ju + Bu),
(134)
where  > 0, and J denotes the duality map of X. Since A is maximal
monotone, we have R(A + tJ) = X..
(II) Galerkin method. Now apply the proof idea of Theorem 32A to (134).
(III) Study the limiting process t  o.
A detailed proof can be found in Browder (1968(76, M p. 92 and in Kluge
(1979, M), p. 195.
32.5. A special class of maximal monotone nuJppings. Let
A: X -+ 2%.
be monotone and hemicontinuous on the real reflexive B.spacc X, and suppose
that.. for each u eX.. the set Au is nonempty, closed. and convex in X.. Show
that A is maximal monotone.
Hint: Use the monotonicity trick from Problem 32.2b.
32.6. Properties of maximal monotone mappings. Let the map
A: X -+ 2 x .

Problems
915
be maximal monotone on the real reflexive 8-space X. We summarize a
number of important properties of A.
(a) Let (14",14:) E A for all n e f\J. If
14"  u in X and
then (14, Ii.) EA.
(b) Let fUll' 14:) E A for all II E . If
u.u. in X.
"
as n..... oc,
14" --A Il in J(
and
u. -+ 14* in X *
"
as n..... 'X,
then (14,14.) E A.
(c) For each 14 EX, the set Au is convex and weakly closed.
(d) The map A is demicontinuous and and locally bounded on int D(A).
(e)* The sets int D(A), D(A) , and R(A ) are convex.
(f)* If int D(A)  0, then int D (A) = D( A) .
(g) If D(A) = X, then A is pseudomonotone.
(h) The inverse map A -I: X.  2 x is maximal monotone.
(i) The map A is surjective iff A -I is locally bounded.
(J). Theorem of Zarantonello (1973) and Kenderov (1976). If intD(A):# 0,
then there exists a dense subset S of D(A) such that the map A is single-
valued on S.
The set S is the intersection of a countable family of open sets. If
dim X < ('$.), then the set D(A) - S has Lebesgue measure zero.
This theorem tells us that multivalued maximal monotone mappings
are single-valued up to a Usmall" singular set D(A) - S.
Hint: Ad(a), (b). The map A is monotone. "rom
( 14. - t'. U - V ) > 0
" ' " -
for all (v, v.) E A, n E ,
it follo's that (14. - t'.,u - v)  0 for all (t'.I'.) E A, and hence (14,14.) E A.
Ad(c), (h), (i). Cr. Propositions 32.5 and 32.6, and Theorem 32.G.
Ad(d). The local boundedness of A follows from Proposition 32.33. The
demicontinuity of A follows similarly as in the proof of Proposition 26.4. Cf.
Kluge (1979, M), p. 33.
Ad(e), (f). Cf. Rockafellar (1968), (1969).
Ad(g). Cr. Pascali and Sburlan (1978, M), p. 106. Use (d).
Ad(j). Cr. Nirenberg (1974, L), p. 183, and Deimling (1985, M), p. 292.
32.7. Characterization of ma;mal monotone mappings by skew-s}'mmetric saddle
.(unctions.
32.7a. Definitions. A function f: X  [ -00, 00] on the real 8-space X is conrex iff
the epigraph,
epi f = {(u, t) E X x R:f(u) S; t},
is a convex set (see Section 47.1). Let M(u) be the set of all continuous affine
minorants of f at the point 14, i.e., 9 E M(u) iff
f(v) > g(t') for all v E X,
where g = u. + const with u. E X.. The closure of f is defined by
(cl f)(u) = sup g(u).
'E .'1c..)
In particular, if M(u) = 0, then (cl f)(u) = - oc.

916
32. Maximal Monotone Mappings
The function f: X -. [ -. J)] is caJled concat"e iff - f is convex.
By a sken'-S)tmmelric saddle function
L:X x X-.[-oo.oc].
we understand a function which has the following properties:
(i) 1It-+ L(u. v) is concave on X for each VEX.
(ii) Ii  L(u, t f ) is convex on X for each II eX.
(iii) el:! L(u, v) = ell ( - L( [', u)) for all u, veX. \\'here cl i refers to the ith variable.
32.7b.* A general,Ilforem. Let X be a real renexi\'e B-space. Let L in (135) be a
skew-symmetric saddle function. We define the map
A L : X -+ 2 x .
( 135)
through (u, u*) E A L iff
u*(h) S L(u, U + II)
for all hEX.
Show the follo\\'ing:
(i) The map A L is monotone.
(ii) If el 2 14 = L, then A is maximal monotone.
(iii) Conversely, each maximal monotone mapping A: X .... 2 x . can be
obtained this way. i.e.. there exists a skc\\'-symmetric saddle function
I: X x X .... [ -oc, OCI] such that el2 L = Land A L :; A.
Moreover. we have
o € All
iff (u, u) is a saddle point of L.
This theorem alloYt.s a pscudovariational approach to maximal monotone
mappings. Moreo\'er. this theorem explains Yt'hy maximal monotone map-
pings behave, in many respects, like subgradients of con't'ex functions.
32.7c. Simple example. Let f: X .... R be convex. \Vc set
L(rl, t') = f{l') - feu).
Then L: X x X -. R is a skew-symmetric saddle function, and
At = of.
In particular. if J- is CJ, then the operator
A L == .('
is maximal monotone on X, since A L is monotone and continuous.
Hint: Cf. Krauss (198Sb). (1986). These papers also contain interesting appli-
cations of this approach.
32.8.. Proqf oj. The(Jrem 32.P.
Hint: The proof of Theorem 32.P(a) proceeds similarly to the proof of
Theorem 31.A. In this connection. use the properties of the Yosida approxi-
",atian for multivalued maximal monotone operators (d. Chapter 55).
Let {5(1)} be a nonexpansive scmigroup on the nonempty closed convex
subset C of the real H-space X. In order to prove Theorem 32.P(b). let
B I . u - S(h)u
u = 1m h '
'-+0

Problems
917
where u e D(B) iff the right-hand limit exists. The operator B is monotone.
Let A be a maximal monotone extension of B. Hence D(A)  co D(B)  c.
Aocording to Theorem 32.P(a). the operator A gencrates a noncxpansivc
semigroup {SA(I)} by solving u' + Au 30 and letting U(l) = SA(t)U(O). Show
that S(t) = SA(t) for all t  o.
A detailed proof can be Cound in Breris (1973. L), p. 114.
32.9.- The range of sum operators. We assume:
(HI) The linear operator A: D(A) s; X ... X is graph closed on the real H.
space X. where D(A) is dense in X and R(A) is closed.
(H2) Let N(A) = .IV(A*). where A- denotes the adjoint operator. This is equi-
valent to R(A) = N(A)l. Consequently, the operator A: D(A) n R(A)-+
R(A) is bijective.
(H3) The inverse operator A-I: R(A) -+ D(A) ('"\ R(A) is compact.
(H4) lt 2 be the largest positive number such that
I
(Aulu)  - -IiAuIl 2
2
for all u e D(A).
In particular, if (Aulu)  0 for all u e D(A), then we set  = + 00.
In this connection. obscn'c the following. We have the orthogonal
decomposition
X :s N(A) Ef) R(A).
Lct P be the orthogonal projection operator from X onto R(A). f-"'rom (H3) it
follo\\'s that there is a number c > 0 such that, for all u e D(A), \\'e obtain
"AuQ = IIAPull  ell Pull.
and hence
(Aulu) = (AuIPu)  -IiAuli IIPuj  -c- I i AuC 2 .
Consequcntly, condition (H4) makes sense.
(H5) The nonlinear operator B: X -+ X is monotone and hemicontinuous.
32.9a. Assume (H I) through (H5) and prove the following four statements:
(i) If R(B) is bounded, then
R(A + B)  R(A) + co R(B) (136)
(see Definition 32.45).
(ii) Suppose that there is a number fJ with 0 < fJ <  such that
I
(Bu - Bvlu)  fJ IIBufi2 - 7(V)
where the real number I' depends only on v. Then rclation (136) holds. If,
in addition, N(A) s; R(B) (e.. B is weakly coercive), then
for aJl u, veX t
R(A + B) = X.
(iii) Suppose that If + J.l: X -+ X is bijective for some fixed.A. > 0, and suppose
that
. IIBu - Aull 0
lam = .
h. - 0: II u II
( 137)
Then R(A + B) = X.

918
32. Maximal Monotone Mappings
(i\') Suppose that R(B) c: X (e.g., B is weakly coercive). and suppose that there
is a number II with 0 < fJ < eX such that
1
(Bu - Bvlu - v) > fJ IIBu - Bvn 2
for all u, veX.
Then R(A + B) = X. For each be X, the equation
Au + Bu = b, u eX"
(138)
has a unique solution mod JY(A). i.e., ifu and ii are solutions of(138), then
u - ii E N(A). If B: X -+ X is bijective (e.g., B is weakly coercive and
strictly monotone then the solution of (138) is unique.
32.9b. Assume (H 1) through (HS) and assume that B = lp', where the function lp:
X -+ R is convex, continuous, and G-differentiable. Prove the following two
statements:
(i) If
J o- II Bu II 2
1m < -,
1_1- 2: ,1uU 2
then R(A + B)  R(A) + co R(B).
(ii) If R(B) = X (e.g., B is weakly coercive), and if there is a number fJ with
o < p < eX such that
(Bu - Bvlu - t') < fJllu - vll 2 for all u. veX"
then R(A + B)  R(A) + co R(B).
In (ii). the compactness condition (H3) drops out.
Hint: cr. Brezis and Nirenberg (1978). In this paper, one also finds appli.
cations to nonlinear elliptic, parabolic, and hyperbolic equations.
References to the Literature
Classical works: Minty (1962), (1963), Browder (1963), (1968 (1968a), Rockafellar
(1966), (1968), (1969 (1910), Brezis and Haraux (1976).
Monograph on maximal monotone operators in H-spaces: Brezis (1973
Monographs on maximal monotone operators in B-spaces: Browder (1968/76
Pascali and Sburlan (1978). Kluge (1979).
Range of sum operators: Brezis and Haraux (1916), Brezis and Nirenberg (1978).
Single-valuedncss of maximal monotone mappings: Zarantonello (1913), Kendero\'
(1976 Fitzpatrick (1971). Deimling (1985, M).
Maximal monotone mappings and skew-symmetric saddle functions: Krauss
(198Sb). (1986

CHAPTER 33
Second-Order Evolution Equations
and the Galerkin Method
I attended the Technological Institute in Graz (Austria). One of the most worthy
representatives of structural engineering there gave a lecture on the most reason.
able installations of 100 furnishings. One day, during a lecture. he drew a circle
on the seat of a chair with chalk. Then he sat down, stood up again, and turned
his colossal backside towards us. Thus he showed us the most convenient
choice of the toilet seat. This experience also contributed to me devoting myself
to the pure science of mathematics.
Wilhelm Blaschke (1957)
Two men travel in a balloon above the earth. "Where are weT', asks one of them.
Thereupon the other thinks for a long time and then answers: "We are in a basket
under a nying balloon."
This must have been a mathematician, because he has thought for such a long
tim the answer is correct and useless.
F olclorc
At the beginning of this century, Dcdekind (1831-1916) opened a calender and
read: "Richard Dedekind. Died in Braunschweig on September 4, 1899."
Dedekind then wrote to the publisher of the calender: "Dear Sir. Please allow
me to draw your attention to the circumstance that the date of my death is in
error at least as far as the year is concerned."
Folclore
One day a man came to Bertrand Russell (1872-1970) and asked him: "You say
that a wrong hypothesis implies everything. Please, prove to me from a wrong
hypothesis that I am the Emperor of Japan." "Well," Russell said,
 = I implies that 1 = 2."
"The Emperor of Japan and you arc two, two equals one, and we are done."
F olclore
In order to solve nonlinear second-order evolution equations of the form
(E)
u" + B(I, u. u') = 0,
919

920
33. Second-Order Evolution Equations and the Galerkin Method
one can reduce them to first-order evolution equations. In this connection,
one has the following t\VO possibilities:
(i) If we set
u(t) = f v(s) ds,
then \VC obtain from (E) the equation
v' + 8(t, f V(S)dS,V) = o.
(ii) If \ve set
,
v = u,
then we obtain from (E) the system
v' + B(l, u, v) = 0,
u' - v = o.
Both methods will be used in this chapter. By using (i), Theorem 33.A in
Section 33.3 justifies the Galerkin method for a class of second-order evolution
equations which contain monotone operators. The proof of Theorem 33.A
will be based on Theorem 32.E which follows from the theory of maximal
monotone operators. In Section 33.5 we consider applications of Theorem
33.A to a class of quasi-linear hyperbolic differential equations, which are
nonlinear with respect to the first time derivative.
In Sections 33.6- 33.11,. we study the following topics:
(a) Symmetric hyperbolic systems and systems of conservation laws.
(b) Formation of shocks by intersection of characteristics.
(c) General blowing-up effects.
(d) Blow-up of solutions for semilinear wave equations.
(e) Global solutions for semilinear wave equations and the semilinear Klein-
Gordon equation.
(f) Generalized (viscosity) solutions of the Hamilton-Jacobi equation.
These topics will be investigated in greater detail in Part V in connection
with basic problems in mathematical physics. For example, in Chapter 83 we
\vill explain the fundamental role which is played by quasi-linear symmetric
hyperbolic systems in mathematical physics.
Roughly speaking, all processes in nature which correspond to the propa-
gation of ',,'Qt,es lead to hyperbolic partial differential equations. This explains
the importance of such equations. The mathematical theory for hyperbolic
equations encounters a number of typical difficulties which are mainly based
on the possible formation of slaocks (the appearance of discontinuites after a
finite time despite smooth initial data) and on blo\\';ng-up effects. This will be
discussed in Section 33.7.

33.2. Equivalent Formulations of the Original Problem
921
33.1. The Original Problem
Let" V c: H s; V." be an evolution triple. We want to solve the following
initial value problem:
u"(t) + A(t)u'(t) + Lu(t) = b(l)
for almost all t E ]0, T[.
u(O) = u' (0) = 0, ( I )
11 E C( [0. T], V), U' E W p l (0, T; V, H), 2  p < oc.
For each, E ]0, T[, we are given h(l) E H and the operators
L, A ( t): V -+ V..
Moreover, let 0 < T < 00 and q-I + p-I = I. The precise assumptions for
problem (1) will be formulated in Section 33.3. We set
X = Lp(O. T; V).
Then ,,. = Lq(O, T: V*). Since I < q < 2 < p,
X c L 2 (0, T; H) e X..
Recall that the condition u' e W p l (0, T; V, H) means that
u'eX and u"eX*.
Let u' E Wi (0, T; v, H). After changing the function t  u'(t) on a subset of
[0, T] of measure zero, if necessary, we obtain a uniquely determined function
u' E C( [0, T], H),
i.e., u': [0, T] -+ H is continuous. The initial condition 14'(0) = 0 is to be
understood in this sense.
33.2. Equivalent Formulations of
the Original Problem
33.2a. The Functional Equation
Problem (I) is equivalent to the following equation. For alll E V and almost
all t e ]0. T[, let
d 2
dt 2 (U(t)II')H + a(t; u'(t), v) + c(u(t), I') = b(t; I'),
u(O) = u'(O) = 0,
U E C([O, T]. V), u' e Wpl(O T: V,H),
(2)

922
33. Second-Order Evolution Equations and the GaJerkin Method
where d 2 jdt 2 denotes the second generalized derivative on ]0, T[, and we set
aCt; v, w) = (A(t)v, w)."
c(v; \v) = (Lv, \v)y,
bet; v) = (b(t), v)., for all v, ,,, e 
Indeed, it follows from (1) that
(rl"(t), v)y + (A(t)u'(t), v)y + (Lu(t), v)y = (b(l), v)v
for all v E II:
(2.)
According to Proposition 23.20,
d 2
(u"(t). v)v = dt 2 (u(t v)v
for all v e JI:
Furthermore, since U(I), v e  we obtain that
(u(t), v)., = (u(t)IV)H.
In Section 33.5, we shall show that initial value problems for hyperbolic
differential equations can be easily formulated in the form (2).
33.2b. The Galerkin Method
Let {\\'1' \"2'.. .} be a basis in JI: We set
"
u,,(t) = L Cb(t)W t .
':=II
Motivated by (2*), we define the Galerkin equations in the following way. For
j = 1,..., n and almost allt E ]0, T[, we consider:
(u:(t), ,,)v + (A(I)ll(t), wj)v + (Lull(t), wJ)y = (b(l), '''j)v,
u,,(O) = u(O) = 0, (3)
Un e C([O, T], ), u e W p 1 (O, T; Jt;., Hit).
Here, we set
 = H" = span { 'VI' . . . , "',,},
and we equip  and H" with the norm of V and the scalar product of H,
respectively.
Using (2), the Galerkin equations (3) may be written in the following
equivalent form:
t c;'(t)("'.J WJ)H + a ( t; t C t "(I)"'4;. "'J ) + C ( t Cb(t)W4;t \ ) = b(t; "J)'
i=1 '''1 i-1
(4)
c.;..(O) = c;"(O) = 0, j = I,..., n,
for almost all t e ]0, T[.

33.3. The Existence Theorem
923
In Section 33.5, we shall show that the Galerkin method for quasi-linear
hyperbolic differential equations leads directly to (4). Equation (4) represents
a second-order system of ordinary differential equations. Since "'1' . . . , w. are
linearly independent, we have
dct«w.1 "j)8) :t- 0, j, k = I, . . . , n,
and hence equation (4) can be solved for the second time-derivatives c;".
In the standard textbooks of numerical analysi one finds well-elaborated
methods for solving equation (4) on computers.
33.2c. The Equivalent Operator Equation
The original problem (1) is equivalent to the following equation:
14" + Au' + Lu = b,
r4(0) = u'(O) = 0,
U E C( [0, T], V), U' E W,l (0, T:  H),
where we set X = Lp(O, T; V) and the operators
A, L: X  X.
(S)
are defined through
(Au)(t) = A(t)u(t)
and
(Lu)(t) = Lr4(t)
for all t E ]0, T[.
Here, let b e L 2 (0, T; H) be given. Hence b EX..
33.3. The Existence Theorem
We make the following assumptions:
(H I) Let .. V c: H s; V." be an evolution triple with dim V = 00. Let
{W I ,\V2""} be a basis in  Moreover, let 2  p < 00, q-I + p-l = I,
and 0 < T < 00.
(H2) For each t E ]0, T[, the operator
A(t): V.-. V.
is monotone and hemicontinuous with A(t)(O) = O.
(H3) For each t e ]0, T[, the operator A(t) is coercive, i.e., there are constants
C 1 > 0 and C2 > 0 such that
(A(t)v, v).,  clllvn - C2 for all ve J': t e ]0, T[.
(H4) For each t e ]0, T[, the operator A(t) is bounded, i.e., there exists a
nonnegative function C3 e Lq(O, T) and a constant C4 > 0 such that
UA(t)vllv.  C3(t) + c4lJvllq for all v e  t E ]0, T[.

924
33. Second-Order Evolution Equations and the Galerkin Method
(HS) The map t.-+ A(t) is weakly measurable, i.e., for each 14, v E V. the function
t...... (A(t)u. v)v
is measurable on ]0, T[.
(H6) The operator L: V ..... V. is linear, s}'mmetric, and strongly monotone.
(H7) Let b e L 2 (O, T: H) be given.
Theorem 33.A. AssunJe (HI) throug" (H7). Then:
(a) Existence and uniqueness. The original proble1)1 (I) has a uniqrle solution
u.
(b) Convergence of the Galerkin method. For each n e N, the Galerkin equa-
lion (3) has a Ilniqlle solution u... As n  00, the sequence (u ll ) converges to
the solution u oJ tile original problem. More precisel}', we have that
max lIu..(t) -- II(t)lIv -+ 0
OrT
as IJ -. .
and
max lIu(t) - U'(I)U H  0
OsrsT
as n -+ oc.
(c) Equivalent operator equation. The origilUlI problem (I) is equivalent to tile
operator equation (5), \vhere the operator A: X  X. ;s nwlJotone, IJenJi-
continuous, coercive. and bounded. Moreover, the operator L: X ... X. is
linear. s}1mnJelric, and strong/:)' monotone.
Applications to hyperbolic differential equations will be considered in
Section 33.S.
33.4. Proof of the Existence Theorem
We \vill use Theorem 32.E. To simplify notation, we write
IIvll = IIvll v' Ivl = III'U H , (ulv) = (ulv)n,
(u, v) = (u, v)y.
let us recall that
(ulv) = (u, v)
for all u e H, v E V,
according to (23.17).
Step I: Existence proof for the original problem (I).
We set (AI4)(t) = (Au)(t) and (Lu)(t) = LU(I). The proof of Theorem 30.A
shows that the operator
A: X  X*
is monotone, helnicontinuou coercive, and bounded. Obviously, the original

33.4. Proof of the Existence Theorem
925
problem (I) is equivalent to problem (5). According to Theorem 32.E, problem
(5) possesses a solution.
Step 2: Uniqueness proof for (1).
We set
(Sv)(t) = f v(s) ds.
The proof of Theorem 32.E shows that problem (5) is equivalent to the
first-order evolution equation
v' + Av + LSv = h,
Moreover by (32.80
v e W p 1 (0, T; V, H)
v(O) == o.
(6)
f (L(Sv)(s). v(s» ds  O.
for all t e [0, T] and all v e W p 1 (O, T: V, H). Using integration by parts, the
same argument as in the uniqueness proof for Theorem 30.A yields the
unique solvability of (6). Thus, the original problem (1) has a unique
solution.
Step 3: Unique solvability of the Galerkin equation (3).
For v E V and fixed t E ]0, T[, we consider the following functionals on V:
A(t)v, Lv, b(t) e V*.
(7)
The restrictions of these functionals to the linear subspace Jt;. of V are denoted
by
A,,(r)v, L"v, b,,(r) E Jt;.*, (7*)
respectively. Using this notation, the Galerkin equation (3) can be written in
the following form:
u:(t) + A"(t)u(t) + L.u,,(t) == bll(t) for almost all t e ]0, T[,
u,,(O) == u(O) == 0, (8)
". e C( [0, T], Jt;.), u E W,I (0, T; Jt;., H II ).
Replacing V and H with  and H", respectively, the same proof as for (1)
shows that problem (8) has a unique solution.
Step 4: A priori estimates for the Galerkin solutions.
For each n e N we have Jt;. == HII according to the definition of these spaces.
Since dim  < 00, we may identify V". with V n , i.e.,
 == H" == ..
Moreover, since all norms are equivalent on rinite-dimensional a-spaces, we
obtain that
1 (0, T; Jt;. H,,) c: W p l (0, T;  H).

926
33. Second-Order Evolution Equations and the Galerkin Method
Lemma 33.1. For all n E N and allt E [0, T], we have the following estimates:
I u(t)1  const,
II u II x  const,
lIulI(t)1I  const,
IIAulIx. < const,
II LUll II x. < const.
(9)
( 10)
( II)
( 12)
( 13)
PROOF. The proofs of (9), (10), and (II) will be given in Problem 33.1. Since the
operators A, L: X ..... X. are bounded, the estimates (12) and (13) follow from
(10) and (II), respectively. 0
Step 5: Convergence of the Galerkin method.
Let U denote the solution of the original problem (I). Then u' E W p l (0, T;  H).
(I) Preparations. We will use the following approximation argument.
Lemma 33.2. For each n E N, there is a polynomial q,,: [0, T] ..... V" with coeffi-
cients in V" such that q,,(O) = 0 and:
II q  - u' II x + II q: - u" II x. ..... 0 as n..... 00, ( 14)
q" ..... U in C( [0, T], V) as n..... 00, (15)
q ..... u' in C( [0, T], H) as n..... 00. (16)
In particular, it follows from (16) that
q(O) ..... u'(O) in H as n -+ 00. (17)
The proof of Lemma 33.2 will be given in Problem 32.2.
Lemma 33.3. For all n E N and all t E [0, T], we have
t (Au - Au',u - u')ds > 0, (18)
2 f (Lu" - Lu,u - u')ds = (Lu,,(t) - Lu(t),u,,(t) - u(t)
> cllu,,(t) - u(t)1I 2 , (19)
where c > 0 ;s fixed.
PROOF. Ad( 18). This follows from the monotonicity of A (s) for all s.
Ad(19). This follows from (32.79) and from the strong monotonicity of L.
o

33.4. Proof of the Existence Theorem
927
(II) They key relation. Using the original problem (1), UN + Au' + Lu = b,
and the Galerkin equation (3), integration by parts yields the following key
relation:
A" d.!.r it U(l) - q(t)12 - ! lu(O) - q(O)12
= f (u; - q:,u - q>ds
= f (- Au - LU n + (un + Au' + Lu) - q;, u - u' + u' - q > ds
= - f (Au - Au',u - u') + (Lull - Lu,u - u')ds
+ f (u" - q:,u - q) - (Arl - Au' + Lu. - Lu.u' - q>ds
< f (u" - q:. u - q) - (Au - Au' + LUll - Lu, u' - q) ds
S Jlu N - q:llx.llu - qlIx
+ IIA,, - Au' + LU n - Lullx.lfu' - qlIx dd '"
(20)
where
b n -. 0
as n -. 00.
(20*)
by Lemmas 33.1 and 33.2. In particular, note that
lIu N - Q:l1 x . -.0 and lIu' - qlIx -t 0
and note that the following sequences
( II U  II x), ( II q  " x ), (II A u  II x. ), ( 11 Lu" " x. )
as n... 00,
arc bounded.
(III) We want to show that
u ..... u' in C([O, T], H) as n -+ 00.
Indeed. from u(O) = 0, u'(O) = 0, and
q(O) -+ u'(O) in H as n -. 00
(21)
it follows that
u - q .... 0 in C([O, T], H) as n..... 00,
according to the key relation (20), (20.). By (16), we obtain (21).
(IV) We want to show that
u" --. 14 in C( [0, TJ, V)
as n.... 00.
(22)

928
33. Second-Order Evolution Equations and the Galerkin Method
Indeed, consider the key relation (20). (20*) and notice that
4"  0
as n -+ OCI
and
J (u" - q:.u - q> - <Au - A," + Lu" - LII.u' - q>ds
S bIt -t 0 as n -. 00.
Thus. it follows from 14emma 33.3 and (20) that
max f' (LII" - Lrl. u - u' > ds - 0
os's T Jo
as n -+ 00.
Again by Lemma 33.3.
c f'
2 I1u ,,(I) - u(/)1I 2 < J 0 <Lull - LII. u - u') ds.
This yields (22).
o
33.5. Application to Quasi-Linear Hyperbolic
Differential Equations
Let QT = G x ]0. T[. We consider the following boundary-initial value
problem corresponding to a wave equation with nonlinear friction:
N
14" - Au - > D,((IDurI1)DiU,) = f on QT'

u(x, t) = 0 on oG x [0. T],
14 (x, 0) = u,(x,O) = 0 on G.
(23)
We make the following assumptions:
(H 1) Let G be a bounded region in R N , N > I. Let x = (.,..., N)' D i = alaf'
We set
."1
IDv(x)1 2 = L ID,v(X),2.
i=1
(H2) Let 0 < T < 00 and fe L 1 (QT).
(H3) The function 1%: [0, oc [ -+ [0, oo[ is continuous. There are positive con-
stants M and d such that
o  (a2) S M for all (1  O.
a(t 2 )t - a«(11)(1  d(t - 0') for all real T  (1  o.
(H4) Let {"'.. \\'2"..} be a basis in W 2 1 (G).

33.5. Application to QuasI-Linear Hyperbolic Differential Equations
929
Definition 33.4. Let V = W 2 1 (G) and H = L 2 (G). The generali:ed problem
corresponding to (23) reads as follows. We are given f E L 2 (QT)' We seek a
function t  U(I) such that, for alll' E V and almost all t E ]0, T[,
d 2
dI 2 (u(r) l t')" + a(u'(I), t') + C(U(I), v) = h(l; t'),
u(O) = 14'(0) = 0,
U E C( [0, TJ, J/), u' E W 2 1 (0, T; JI, H)..
(24)
where we set
f. ,-W
(U,t') = G j :x(IDuI 2 )D j uD j t'dx,
f. .Itt/ f.
c(u, (') = .L Vi uD; l' dx, b(l, v) = f(x, t)r'(x) dx,
G 1=1 G
(ult')" = L uvdx,
for all II, I' E V and aliI E ]0, T[. Here, d 2 jdt 2 is to be understood as a second
generalized derivative on ]0, T[.
Problem (24) follows formally from the original problem by multiplying (23)
with r E Co(G) and by using subsequent integration by parts.
To formulate the Galerkin method, we set
"
u" ( t) = L C tIt ( t) "', .
t=1
Then, for each n E N, the GalerkiP1 equation reads as follows:
t C ',,( 1)( w, I j)" + a ( t C ,,( I) W" Wj ) + C ( t CAlI ( r) w,. \ )
'=1 k=1 k=1
= b(t, "j) on ]0, T[. (25)
Cj,,(O) = c 1 ,,(0) = 0,
j = 1,..., n.
Proposition 33.5. Assume (H 1) through (H4). Then:
(a) fOor each IE L 2 (QT)' the generalized problem (24) correspondi,zg to (23) has
a unique solution u.
(b) For each n E N, Ihe Galerkin equat;oP1 (25) has a u'1;que solution fl" \\'itll
u", u E L 2 (0, T; V).
As n -+ ex:, the sequence (U,.) conl'erges 10 u. More precisely, \\'e hal'e
f. N
max lu,,(x. t) - 14(:<, t)1 2 + L ID;u,,(x, t) - D;u(x, 1)1 2 dx -+ 0,
OsrsT Ci i=1

930
33. Second-Order Evolution Equations and the Galerkin Method
and
max f. I D,u,,(x, t) - D,u(x, e)1 2 dx -. 0
O'ST G
,,,here D, = c/ot.
as n -. 00,
PROOf. According to (25.87*), there exists an operator A: V --t> V* such that
(Au, v)y = a(u, v) for all u, v E V.
\\'here A is continuous, strongly monotone, and bounded. By Section 22.2a,
there exists an operator L: V -+ V. such that
(Lu, v)., = c(u, v) for all u, v e J1:
where L is linear, symmetric, and strongly monotone.
Finally, it follows from f E L 2 (Qr) that there exists a b E L 2 (O, T; H) such
that
(b(t), v) = b(t; v)
for aU v e V,
and almost aile e ]0, T[, according to Example 23.4.
The assertion now follows from Theorem 33.A.
o
33.6. Strong Monotonicity, Systems of Conservation
Laws, and Quasi-Linear Symmetric
Hyperbolic Systems
The part of my work that was most strongly innuenccd by von Neumann's
work concerned spectral theory and partial differential equations. In it. I ha'..e
restricted myself to linear equations and also to systems of first order. The latter
restriction is convenient and not severe, since equations of higher order can-in
general-be reduced to systems of the first order.
I have also always restricted myself to dealing with symmetric systems. The
reason for this restriction \\'as that almost all nondegenerate panial differential
equations of classical mathematical physics appear either naturally as symmetric
equations, or can be reduced to symmetric equations. Also, fortunately. it is
much easier to sol\'e symmetric equations than nons)'mmetric ones.
Kurt Otto Friedrichs (1980)
We consider the following initial value problem for a system of conservation
laws:
.'1
 ,..
u, + DJfj(u) = Sex, t, u) on R x [0, T],
:1
14(X,0) = uo(x) on R N ,
where II = (u1,...,u.v) and x = (I'...'.v) with Dj = a/aj. Note that fj(u) is
(26)

33.6. Strong Monotonicity. S)'stcms of Conserva(ion Laws
931
a real (M x J\I)-matrix, and Sex, t, u) is a real JW -vector. We assume:
(H 1) Let Fj and S be smooth, i.e.
Fj e CW(R M , R M x RM), S e CXI(IRH+.V+I, R'V),
wherej = I, ..., Nand N, M  t.
(H2) Let Uo e W 2 '" be given with m > 1 + N /2.
Here, we set W 2 '" = W;'(R N , RM), i.e., all the components of U o belong
to the Sobolev space W 2 "'(R N ).
(H3) Let uo(x) e U o for all x e R', "'here U o is a bounded open subset of a
given open set U in R N with U o c U.
(H4) The linearized system
It
u, + L Aj(v)Dju = 0
JSI
with Aj(v) = Fj(v) is symmetric h}tperbolic on U, i.e., there exists a C"XJ.
matrix function
u...... B(u)
from U into R'" x AM such that the matrices
B(u)
and
B(u)AJ(u)
are symmetric for all j, and for each compact subset C of U, there is a
constant c > 0 such that
(B(u)xlx)  clxl 2
for aU u e C, x e R"',
i.c., B(u) is strongly monotone (uniformly with respect to compact u-sets).
Theorem 33.8 (Majda (1984». Assume (HI) through (H4). Then there is a time
interval [0, T] ,,,ith T > 0, so ll,at tI,e original problem (26) has a unique classical
solution
u e C 1 (RAV x [0, T]t (JiM)
\vith the property that u(x, I) lies in a compact subset of U for all x E AN,
I E [0, T].
J\1oreover, we have
U e C([O, T], W z "') n C 1 ([O, T], W 2 ",-I),
and T depends only 0'1 II Uo II M.;' and the set V o .
The proof of this important theorem can be based on the iteration method
of Theorem 21.H (see Majda (1984, L), p. 30) or on the Galerkin method of
Theorem 30.8 aloog the lines of the proof for the generalized Korteweg-
de Vries equation in Chapter 30. In Chapter 83 we will prove a general
existence theorem for nonlinear symmetric h}tperbolic systems which con-

932
33. Second-Order Evolution Equations and the Galerkln Method
tains Theorem 33.8 above as a special case. In Chapter 83 we will also discuss
in detail the physical background of equation (26) and the physical meaning
of the corresponding a priori estimates (energy estimates). Those estimates
concern the quantity
£(1) = fR' (B(u)ulu) dx
which frequently corresponds to the physical energy of the system. Many
important problems in mathematical physics, which describe the propagation
of waves, can be reduced either to problem (26) or to more general quasi-linear
symmetric hyperbolic systems (e.g., the basic equations in fluid dynamics,
gas dynamics, electromagnetism, relativistic quantum mechanics, general
relativity).
If u is a solution of (26), then integration by parts yields
d _d f. u(x, t)dx = - f. f fj(u)njdO + f. S dx
t G i'G};:1 G
(27)
for each bounded region G in [RN with aG E Co. I, where n = (n I , . . . , n N )
denotes the unit normal to aG. As in Example 31.17, relation (27) motivates
the designation "conservation law" for (26).
The theory of linear symmetric hyperbolic systems was created by f'ried-
richs (1954), who discovered the crucial role played by such systems in mathe-
matical physics.
As a special application of Theorem 33.8 to second-order semilinear wave
equations, we consider the following initial value problem:
v" - v = fO(x, t, D. v,. . ., DNv, V" v) on AN x [0, T],
v(x,O) = a(x) on R N , (28)
v,(x,O) = b(x) on R N .
We set ;( = (I'...' N)' DJ = a/aj' and we are looking for a real function
v = v(x. t).
Proposition 33.6 (Semilinear Wave Equation). Let F: R 2N + J -. R be Coo. Then,
for given real functions
a E W 2 ",+1 (R N )
and
b E W 2 "'(R N )
K'ith m > I + N /2, there exists aT> 0 such that the original proble,n (28) has
a unique classical solution v E C 2 (R N X [0, T]).
PROOf:. Let N = 2. The general case proceeds analogously. We set
u = (U 1 ,U2,UJ'U 4 ) = (D 1 v,D 2 v,v"v)

33.6. Strons Monotonicity, Systems of Consen'8tion Laws
933
and "0 = (D 1 a,D 2 a,b,a). Using
(D)v), = DJv"
we obtain from (28) the following first-order system:
(u I ), - D'"3 = 0,
(U2), - D2"3 = 0,
( 14 3), - D 1 u 1 - D2U2 = F(x. t, u),
(U4), = U3.
(29*)
This can be written as
u, + AID!u + A 2 D 2 11 = S on R.- I x [0, T],
u(x,O) = uo(x) on AN,
(29)
where
0 0 -1 0 0 0 0 0
A I = 0 0 0 0 A 2 = 0 0 -I 0
-1 0 0 o · 0 -1 0 o '
\ 0 0 0 0 0 0 0 0
0
s= 0
F(x, t. 14)
"3
Since the matrices A I and A 2 are symmetric, the system (29) is symmetric
hyperbolic with B = 1, the unit matrix.
Obviously. the original problem (28) is equivalent to (29). In this connection,
observe the following. Suppose that u is a classical solution of (29). Letting
v = U4, it follows from (29*) that
(DJv), = DJ U 3 = (u), for t  0,
and DJv = uJ for t = O. Hence DJv = u J for t > o.
The assertion no\\' follows from Theorem 33.8. In this connection, note that
the functions a and b are continuous and bounded on [liNt by the Sobolev
embedding theorems (see A 2 (741». 0
Remark 33.7 (Energy). Since B = It we obtain for the energy the following
well-known classical expression:
E(t) = f, (Bulu)dx = f, f (D j V)2 + v: + v 2 dx.
R R'jl

934
33. Second-Order Evolution Equations and the Galcrkin Method
33.7. Three Important General Phenomena
We want to discuss the following three phenomena:
(i) the appearance of shock waves (discontinuities of the solution);
(ii) the blow-up of solutions;
(iii) the crucial difference between parabolic equations (reaction-diffusion
processes) and hyperbolic equations (wave propagation) with respect to
the smoothness properties of the solutions.
Generally, it is not possible to prove the existence of smooth solutions u of
equation (26) above for all times t > O. This is caused by (i) or (ii). Roughly
speaking, shocks correspond to the collision of waves which propagate with
different speed. Such shocks play a crucial role in gas dynami where they
correspond to discontinuities of physical quantities (e.g. density. pressure.
velocity). The simplest model for this effect will be considered in Example 33.9.
For example, supersonic aircraft cause shock waves which one hears as sharp
cracks. Today, there are no general existence theorems available for the
equations of gas dynamics. The mathematical difficulties are mainly related
to the appearance of complex shock waves.
By a blowing-up effect we understand that the solution goes to infinity after
a finite time. Roughly spcaking such effects occur if too much energy is added
to the system. The simplest model for this will be considered in Example 33.10.
With respect to (iii), roughly speaking, one has the following general
principle:
(P) III contrast to hyperbolic equatiolJs, the solutions of parabolic equations at
tinae t > 0 are, as a rule, smoother than the initial data at time t = O.
In terms of physics, hyperbolic equations describe the propagation of waves,
which does 'lot lead to an increase of smoothness for increasing time. Parabolic
equations describe diffusion processes. Such processes try to remove irregular
situations, which increases the smoothness.
Mathematically, let us look at symmetric hyperbolic systems (Theorem
33.B) and parabolic systems (Theorem 31.C) in R"'. In Theorem 33.8, we need
initial data of the form
"0 E W 2 m (R N , RM m > J + N / (30)
in order to obtain classical C'-solutions. Note that, by the Sobolev embedding
theorems, relation (30) implies that "0 E C I . In Theorem 31.C, we only need
initial data of the form
1 ' R M
Uo e WI' (R, ), p > N,
in order to obtain a classical solution for t > O.
EXAMPLE. 33.8. In order to explain principle (P) above in the simplest way, we
consider the simplest special cases of Theorem 33.8 and Theorem 31.C. First

33.8. The Formation of Shocks
935
we study the hyperbolic equation
u, + crlJ( = 0 x e R, t > 0,
u(x, 0) = uo(x
where c is a positive number. This equation has the solution
u(x, r) = uo(x - cr),
which corresponds to the propagation of a wave with velocity c. Obviously,
the solution u has the same smoothness properties as the initial values Uo. If
1'0 is continuous, but not differentiable, then so is u, etc.
In contrast to (31), we now consider the parabolic equation
(31)
u, - u xx = 0, x e lA, t > 0,
U(X, 0) = uo(x)
(32)
with the solution
I J «X>
u(x,t) = e- tx -,P/4'U o (}7)dy.
2J Tf, I -trJ
Observe that u is CrxJ for t > 0 if Uo is continuous with compact support or,
more generally. Uo e L 1 ( -cc,) (see A 2 (25b».
33.8. The Formation of Shocks
STANDARD EXAt.fPLE 33.9. Let u = u(x, t) be a real function. We consider the
following initial value problem:
u, + f(ul JC = 0,
u(x,O) = l'o(X
which represents the simplest nonlinear example for Theorem 33.8. Let f:
R ... IR be C 2 . It is obvious that (33) is symmetric hyperbolic. However, in this
special case, we can construct classical solutions in an explicit manner by using
the so-called method of characteristics, which will be explained below. We
write (33) in the form
X E IR, I > 0,
(33)
u, + c(u)u x = 0, where c(u) = f'(u). (33*)
(i) Necessar)t condition. Let u = u(x, t) be a C 1 -solution of (33). The solu-
tions x = x(t) of the equation
x'(t) = c(u(x(t), t»
are called characteristics of (33). Differentiation shows that
d
dt u(x(t). t) = uxc(u) + u, = O.
(34)

936
33. Second-Order Evolution Equations and the Galerkin Method
t
-- shock
t
.t
PI P2
(a) (b)
Figure 33.1
Thus, we obtain that:
u is constant along each characteristic.
(35)
From (34) and (35) it follows that the characteristics are straight lines, and
hence they have the form
x = c(uo(p))t + p,
where p is a parameter with x(O) = p.
(ii) Shocks. Suppose that there are two points PI and P2 such that PI < P2
and
(36)
C(UO(PI)) > C(U O (P2 )).
(37)
In this case, the characteristics intersect at a point (x, T) (Fig. 33.1 (a)). Since
u is constant along the characteristics, we obtain that
u(x, T) = UO(PI) and u(x, T) = U O (P2).
But it follows from (37) that UO(PI) =F UO(P2), and hence the classical solution
U can only exist for
t < T.
At least at time t = T, a discontinuity appears. The following observation is
important:
The appearance of discontinuities of the solution does not depend on the
smoothness of the initial data.
The condition (37) is satisfied in most cases. Thus, as a rule, problem (33) does
not have global classical solutions for all t > O. This is a quite remarkable fact.
(iii) Physical interpretation. Regard u(x, t) as the mass density at the point
x at time t. Then equation (33) describes the conservation of mass (see Section
69.1). The characteristics x = x(t) are the trajectories which transport the mass
particles. Discontinuities of the mass density u appear if two trajectories
intersect which transport different densities.

33.9. Blowing-Up Effects
937
(iv) Constrllct;on of classical solutions. Let U o be given as a C 1 -function. By
(35) and (36), a solution of the original problem (33) must have the form
u(x, t) = uo(p),
where p is given through (36). Differentiation shows immediately that tl is a
solution in the case where equation (36) can be solved for p, i.e., we obtain a
classical C I .solution u in each region where the characteristics do not intersect
(Fig. 33.1 (b».
(v) Generalized solutions. We postpone the consideration of generalized
solutions of (33) to Chapter 86, where we will explain the connection with gas
dynamics. At this point, let us only make some general remarks.
(a) By a generalized solution of (33), we understand a solution in the sense of
distributions.
(b) Generally, problem (33) has several generalized solutions.
(c) However, if we add the so-called entropy condition, then it is possible to
prove the existence of a unique global generalized solution for all times
t > o. This has been done by Oleinik (1957).
(d) Roughly speaking, the entropy condition guarantees that the solution does
not violate the second la,,' of thermod)'namics. Thus, among all the possible
generalized solutions of (33), there is precisely one which is meaningful
from the physical point of view. This is a very remarkable fact.
In this connection, we recommend Smoller (1983, M) and the survey article
Lax (1984).
33.9. Blowing-Up Effects
STANDARD EXAMPLE 33.10. Let a e  and let u = II(t) be a solution of the
ordinary differential equation
u' = f(u).
(38)
If f(u) = all, then the solution U(I) = e'" u(O) exists for all times t e IR. However,
if a + 0 and
f(ll) = a(1 + u 2 )«,
 > 1/2,
(39)
then the solutions blo,,' up, i.e., they go to infinity after a finite time. For
example. if « = 1 and a > 0, 11(0) = 0, then
u(t) = tan at.
i.e., the solution exists only in the interval] -x/2a, n/2a[ (Fig. 33.2).
PROOF. If 14 = U(I) is a solution of (38) with (39), then
f. u dv
11(0) a(l + v 2 r = t.

938
33. Second-Ordcr Evolution Equations and the GaJerkin Method
Figure 33.2
Since the integral converges as u ... + 00, the function u = u(t) can only exist
on a finite time interval.
Since f is C., the solution u = u(t) can be continued as long as it remains
bounded, by Section 3.3. Thus, the solution must blow up after a finite time.
D
The same argument justifies the following general principle. Let f: R ... iii
be C 1 with I(u) :F 0 Cor all u e R.
Tile solutions of (38) blow up if f groM's stronger tlaall lilaearlyas u -+ +00.
This principle is a special case of a general result which we will prove below
in Theorem 33.C. As a preparation. we need the following well.known result
on differential inequalities which allows to estimate the life-span of solutions.
We \\'ant to show that
u' S f(u) on [0, T],
Vi = f(v) on [0, T],
u(O)  v(O),
(40)
implies that
u(t)  v(t) on [0. T].
It is important that I is monotone increasing.
(41)
Proposition 33.11 (Differential Inequalities). Let 0 < T < 00, and let f: R ... IR
be a nJOIJotolJe increasing Cl.function i.e., u  v implies feu) s; f(v). Suppose
that ti,e C1.jullctions u, v: [0. T] ... IR satisfy (40).
Thela inequalit}' (41) holds.
Corollal). 33.12. The .ame assertiola remains true if \ve replace" < " ef.,'er),,'here
,,'it'a U > ".

33.9. Blowing-Up FJrccts 939
PROOF. From (40) it follows that
vet) = v(O) + f f(v(s))ds on [O T]
u(t)  u(O) + f f(u(s» ds.
We now consider the iterative method
v ll +. (t) = v(O) + f f(v.(s» ds.
n = 0, I, . . . ,
(41 *)
with Vo = u. Obviously, u S VI on [0, T]. By induction, we obtain that
u(t) S VII(t) on [0, T]
for all n.
By the proof of Theorem 3.A, there is a 1; > 0 such that
V,,(l) ..... v(t) on [0, T 1 ]
as n -+ 00.
This implies
U(l) :s; v(t) on [0, T 1 ].
A simple continuation argument shows that the latter inequality is valid on
[0, T] (cf. Problem 30.2). 0
PROOF OF COROLLARY 33.12. In contrast to the proof above, we now use
u(t)  u(O) + f flues» ds on [O T]
and the iterative method
v.+.(t) = v(O) + ff(v.(S»dS. n = O 1. ....
with Vo = 14. Note that v(O) < u(O), by assumption. Hence, by induction,
vll(t) S; u(t) on [0, T] for all n.
As in the proof above. this implies V(I) S U(I) on [0. T]. 0
We now consider the case where f is only monotone increasing on R+, e.g.,
feu) = I + u 2 .
PropositioD 33.13. Let 0 < T < co and let f: R+ -+ IR+ be a monotone increas-
ing Cl-funcl;o i.e., 0 S u S v implies 0 S feu) s f(v). TheIl:
(a) If the CI-functions u v: [0, T] -+ iii SQliy
u ' S f(u) on [0, T],
Vi = I(v) on [0. TJ,
u(O) s v(O

940
33. Second-Order Evolution Equations and the Galerkin Melhod
and if u(t) > 0 on [0, T], then
u(t)  v(t) on [0, T].
(b) If the C 1 -functions u, v: [0, T] --. IR satisfy
u' > f(u) on [0, T],
v' = f(v) on [0, T],
o  v(O)  u(O),
and if u(t) > 0 OIl [0, T], then
o < v(t)  u(t) on [0, T].
PROOF. We use the same argument as in the proofs of Proposition 33.11 and
Corollary 33.12 above. Note that, in addition, v,,(t) > 0 on [0, T] for all n. This
follows from u(t) > 0 on [0, T] and from v(O) > 0 in (b). 0
More general results on differential inequalities can be found in Walter
(1964, M).
EXAMPLE 33.14 (Quadratic Differential Equations). Let u = u(t) be a solution
of the differential equation
U'(l) = cx(t)U(t)2 on [0, T[,
where 0 < T < 00 and u(O) > o. Suppose that there are numbers p and y such
that
o < p  (t) }' on [0, T[,
and the function tX: [0, 00 [ -+ IR is continuous.
Then, for the life-span of the solution u, we obtain the estimate
(}'U (0) ) - I < T S (pu (0) ) - I .
PROOF. For JJ > 0, the differential equation
Vi = JJl,2,
v(O) = u(O),
has the solution l'li(t) = u(O)/( 1 - Jlu(O)I). By Proposition 33.13,
v_(t)  u(t)  vy(t) on [0, T[. 0
We now want to apply Proposition 33.13 to the differential equation
u ' ( t) = f' ( u ( t), t),
u(O) = Uo
in a real H-space X. We will use estimates of the following form:
t E H,
(42)

33.9. Blo\\'ing-Up Effects
941
(i) Srlblinear growth of F as lIuli .-. <X):
IIF(u,I)II < cUI4U+d forall teR. ueX. (43)
(ii) Srlperlinear gro\vlh of F as lIu II -. 00:
(F(u, t)lu)  0 for all I e Rt U E X,
(F(u, 1)lu)  b lIull" for all t e R, lIuli > lIuoll > o.
(44)
Here, p > 2 and b, c, d are positive numbers.
neorem 33.C (Global Existence and Blo\\'ing Up). LeI Uo E X be give", and let
F: U x [Ii -+ X
be C 1 , where U i. all open subset of the real B-space X ,,'ith Uo E U. JWoreover,
let F be bounded OIl bounded sets. Then:
(a) Existence and uniqueness. TIJere exists a I1Ulximal open inlert'al J = ]S, T[
\vith 0 E J such that the initial t'alue problem (42) has a IInique so/rllion
u = u(t) \vilh U(I) E U for all t e J.
(b) Continuation. The solution u = U(I) of (42) can be uniquely conti"ued
as 10119 as it remains bounded and it does not reach the boulular}' of U
(Fig. 33.3).
(c) Global existence. Let U = X.. \"here X is a real H-space. If (43) holds, then
J = R.
(d) Blowing up. Let U = X. \vhere X is a real H-space. If (44) holds. then
T < co alld
lim lIu(t)1I = 00.
,...T-O
PROOF. Ad(a), (b). cr. Problem 30.2.
Ad(c). Since (u(I)lu(t»' = 2(u'(t)lu(t», we obtain from (42) the key relation
d
dt [11I(t)1I 2 = 2(F(u(l), 1)lu(1)) on J. (45)
I
u U U --+-
I
-- I
",. '"
./ "
/ \ I
( I
I
\ I I
, "
'" ",.
---"
I 1
S T S T
(a) (b)
Figure 33.3

942
33. Second-Order Evolution Equations and the Galerkin Method
If (43) holds, then there is a number a > 0 such that
I(F(u,t)lu)1  cllull 2 + dllull  2- l a(1 + 1114112)
for all t E R, U E X. Hence
d
dl llu(I)1I2  a(l + 1114(1)11 2 ) on J.
Since the majorant equation
v' = a (I + v),
v(O) = II u(O) 11 2 ,
has the solution v(t) = v(O)e OI - I, we obtain from Proposition 33.13 the
a priori estimate
lIu(t)1I 2  v(t)
for t > O.
An analogous argument yields the same estimate for u( - t). By (b), J = R.
Ad(d). Since (F(u, t)lu)  0, we obtain from (45) that lIu(t)1I  1114(0)11 for
t  O. By (44) and (45), there is a number a > 0 such that
d
dt llu(I)1I2 > a(1 + lIu(t)ll4}1/4 for t > 0,
where (J/4 > !. The assertion now follows from Example 33.10 and
Proposition 33.13. D
Finally, we want to use the method of differential inequalities in order to
prove the existence of a unique global solution for the following initial value
problem:
u'(t) = h(u(t), t) on [0, T],
14(0) = 140'
To this end, we consider the following two differential equations:
(E)
(E*)
v'(t) = f(v(t), t) on [0, T],
w'(t) = g(w(t), I) on [0, T],
along with the decisive majorant condition
(M)
g(u, I)  h(u, I) < f(u, t) for all 14 E R..., t E [0, T],
w(O)  U o  v(O).
Our objective is the following inequality:
(I) w(t)  U(I)  v(t) on [0, T].
Recall that R... = {u E R: u > O}.
By definition, a function h: R+ x [0, T] -t R satisfies the condition (L) iff h
is continuous on R... x [0, T] and h is locally Lipschitz continuous with
respect to u, i.e., for each point (u, t) e R.. x [0, T], there exists a neighborhood

33.9. Blowing-Up Effects
943
U in R2 and a constant L  0 such that
Ih(v,s) - h(w,s)1 S Llv - ,vi
for all points (v,s) and <'v,s) in the set U n (R. x [0, T]).
For example, the condition (L) is satisfied if
II: R. x [0, T] -. R is C 1 .
Proposition 33.15 (Majorant Method). The original problem (E) has a unique
C1.solrltion u, and this solution satisfies the inequality (I) provided the follo,v;ng
conditions are satisfied:
(i) Let 0 < T < 00. The t,vo CI-junctions v, w: [0, T] -+ R satisfy the differ-
ential equations (E*).
(ii) The fillictions f, g, h: R+ x [0, T] -+ R+ satisfy the condition (L) and the
majoranl condition (M).
(iii) The functions I and 9 are monotone increasing with respect to the first
variable, i.e.,
I(u, t)  I(v, t)
and
g(u, t)  g(v, t)
for all u, v e R+ and t e [0, T] ,,'illl U  v.
(iv) We are given Uo e R+ such that the majorant condition (M) is satisfied, and
w(O) e R+.
CoroUary 33.16 (General Case). The assertions of Propositioll 33.15 remain
tVllid if we replace R+ with R efI'er)'where in the assumptions (i) through (iv)
above, and in conditions (L) and (M) above.
PROOF OF PROPOSITION 33.1 S. We first prove an a priori estimate for u. Suppose
that u is a C 1 .solution of the equation (E) on the interval [0, S], where
o < s S T. We consider the iterative method
U".l (t) = u(O) + f h(u.(s). s) ds. n = 0, 1. ...,
with uo(t) = u(O) on [0_ S]. By induction t
u,,(t)  0 on [0,5],
since u(O)  0 and 11(u, s) > 0 for aU u > 0, s e [0, T]. By Theorem 3.A, there
is a number So > 0 such that u.(t) -t u(t) OD [0, So] as n -t <X>, and hence
u(t) > 0 on [0, So].
The continuation argument from Problem 30.2 yields u(t) > 0 on [0, S].
Now it is possible to use the same argument as in the proof of Proposition
33.13. Hence we obtain the following a priori estimate for the solution u:
o < ",(t) S u(t) S vet) on [0, S].

944
33. Second.()rdcr Evolution Equations and the Galerkin Method
By Problem 30.2, this a priori estimate guarantees the existence of a unique
solution U for the original problem (E) on the interval [0, S].
Suppose that S < T, otherwise \\'e are done. Applying the argument above
to the initial value problem for the differential equation (E) at the new point
l = S, we obtain the existence of a unique solution u on some larger interval
[0, S + L1] such that 0 S \\1(1) S U(I)  v(t) on [0, S + L1].
Finally, using the continuation argument from Problem 30. we obtain the
existence of a unique solution U for (E) on the interval [0, T] such that
o < v(t) < "(I) S "'(I) on [0, T]. 0
The same way we prove Corollary 33.16.
33.10. Blow-Up of Solutions for Semilinear
Wave Equations
The reason for the improved behavior of solutions of semilinear wave equations
in higher dimensions was beautifully demonstrated by Fritz John (1976) with
the following quotation from Shakespeare, Henry VI:
"Glory is like a circle in the water.
Which never oeaseth to enlarge itself.
Till by broad spreading it disperses to naught."
The case of dimension N = 3 (three space variables), which nature gives pref-
erence, is not onl)' the most important, but also the most challenging.
Sergiu Klaincrman (1983)
In this section we summarize a number of deep results on classical solutions
for semilinear wave equations and semilinear Klein-Gordon equations. We
first consider the semilinear wave equation
Ou = F(u, fI', UN) on R N x [0, T[.
u(.,, 0) == euo(x) and u,(x 0) = £vo(x) on R''I
(46)
for the real function u = u(x, I), where I; denotes a small positive parameter.
Here, x == (l'.. ., .'1)' I = ft'+l' DJ = aloJ' and
1 N
Du = 2 U " - L Dj 2 u.
C )=1
It is well known that the positive number c describes the speed of the pro-
pagation of waves in the case of the linear wave equation (46) with F == O.
Moreover, let u' and un denote the first and second partial derivatives of u,
.
I.e.,
u' = (D. u,. . ., D'+l u)
\Ve assume:
(H I) The function I:: R M -. R is , and F is independent of Ufl. We are given
Uo, Vo e CO>(R''I).
and
u" = (D;D J u),.J=l......tI+l.

33.10. Blow-Up of Solutions for Semilinear Wave Equations
945
(H2) F vanishes together with all its first derivatives at (u, u', u") = (0,0,0).
(H3) F is independent of u.
Definition 33.17. By the life-span 4ri.(t), we understand the supremum over
all T > 0 such that equation (46) has a C-solution u on IR N x [0, T[.
In particular, rit(E;) = 00 means that there exists a global C 7 -solution for
all times I > o.
To begin with, we first formulate the fundamental classical local existence
theorem.
Proposition 33.18 (Schauder (1935)). Assume (H I) and (H2) ';lh N > I for
equation (46). Then there are positit,e constants £0' and A such thaI
A
ri.(E) > -
e
for all E: E ]0, Eo [.
The following example shows that this result is sharp in the case where
N = 1.
EXAMPLE 33.19 (Lax (1964), Klainerman and Majda (1980)). Let N = I and
let
F = f(u)()u)()(.
Here, f: R -+ R is ex with
f(O) = I' (0) = ... = fCi -I )(0) = 0,
l(k)(O) =F 0,
where k > 1. In this case, equation (46) corresponds to the nonlinear string
equation
u" = c 2 ( 1 + f{ux))u xx '
X E R, t > 0,
(46. )
u(x,O) = euo(x), u,(x,O) = evo(x),
where u = u(x, t) describes the shape of the string at time t (Fig. 33.4). Let U o ,
1'0 E CJ(IR) be given. Then the solution of (46.) blows up after the finite time
ri.(e) = O(I;-k) as E; -+ o.
The blow-up occurs in the second derivative of u, i.e., U xx becomes infinite
II
t
Figure 33.4

946
33. Second-Order Evolution Equations and the Galerkin Method
while U x and u, remain bounded. Such blow-ups are typical of shock
formations.
An explicit example will be considered in Problem 33.9.
In the case where N  3, Proposition 33.18 can be improved substantially.
Theorem 33.0 (Klainerman (1985»). Assume (HI), (H2), and (H3). Then there
are positive constants £0 and A such that t for all t E JOt £0[, we obtailJ
'fcri'(£) > {;I£
if N > 3 (global solution),
if N = 3 (almost global solution).
(47)
Corollary 33.20 (Klainerman (1983». Assume (HI), (H2), and (H3), and let
F(II', fI") = O((lu'l + luNI),,) as lu'l + luNI -.0,
,,,here p > 1 + 2/(N - 1) and N  2. Then there is an £0 > 0 SrlclJ tllal, for
each I; e ]0, £0 [, tile origi,JQI problem (46) has a unique global classical solution
U E COO(IJi." x [O.oo[).
The proofs of these important results are based on sharp a priori estimates
for linear and nonlinear wave equations. In this connection, the invariance of
the linear \vave equation under the Lorentz group (cf. Section 75.7) plays a
fundamental role. The estimate ,it(£) > e A/l in the case where N = 3 tells us
that the solution exists for "a long time."
We now study the semilinear Klein-Gordon equation
2 2
,n c F '" )
Ou + ,,2 u = (u, u ,u ,
x e R 3 , t > 0,
u(x,O) = £IIO(X), u,(x,O) = evo(x).
Here. the positive number m can be regarded as the mass of a meson, where
c denotes the velocity of light, and h = h/21t, where h denotes the Planck
quantum of action (cf. Chapter 91 in Part V). It is quite remarkable that, in
contrast to the case m = 0 (wave equation we obtain global solutions if
m > o.
(48)
Theorem 33.E (Klainerman (1985a». Assume (HI), (H2) and let m > O. The"
there is an eo > 0 such that, for each £ E ]0, £0[, problem (48) has a rlniqrle global
classical solution u E CC()(R3 X [0, 00 [), i.e.,
'fc'il(t;) = 00 for all £ e ]0. £0[.
'\lith respect to (48). The solutio" deca}'s as t" 5 .'4 for large times t, i.e.,
const
sup 1 u(x, 1)1 S; s.«4
x  R) 1
for all t > O.

33. J 1. A Look at Generalized Viscosity Solutions
947
The techniques of proof for the above results can also be applied to the
initial value problem for the Einstein equations in general relativity, and to
more general equations of mathematical physics. This can be found in the
monograph by Christodoulou and Klainerman (1990).
33.11. A Look at Generalized Viscosity Solutions
of Hamilton-Jacobi Equations
The notion of viscosity solution of Hamilton-Jacobi equations admits nowhere
differentiable functions and permits a good existence and uniqueness theory. It
is akin to the standard distribution theory, but "integration by parts" is replaced
by .'differentiation by parts" and is done "inside'" the nonlinearity. It is extremely
convenient (as in the distribution theory) for passages to limits. Classical solu-
tions are viscosity solutions. Complete consistency of the classical and viscosity
notions requires that a ,,'iscosity solution which happens to be C 1 will also be a
classical solution. This is indeed the case.
Michael Crandall and Pierre-Louis Lions (1983)
33.11a. Motivation
We consider the Hamilton-Jacobi equation
S, + H(q,t.S q ) = 0
along with the canonical equations
(49)
p= -H.(q,l,p), 4=H,(q,t,p), (SO)
where q, p E IR'''', and the dot denotes the derivative with respect to time I. In
classical mechanics one uses the solutions S = S(q, r) of (49) in order to obtain
solutions q = q(t P = p(t), of the equations of motion (SO). Conversely, one
can solve the partial differential equation (49) by constructing appropriate
families of solutions of the ordinary differential equation (SO This classical
procedure and its physical interpretation will be considered in detail in
Sections 58.24 and 58.25. The Hamilton-Jacobi equation (49) is closely related
to the variational problem:
I 'I L(q(t), 4(t), t)dt = min!,
'0
q(to)=qo, q(t 1 )=ql.
(51)
For fixed qo, to, we set
S(q I' t I) = minimal value of (S I).
Then the function S satisfies equation (49) if the situation is sufficiently regular

948
33. Sccond-Order Evolution Equations and the Galerkin Method
(see Section 37.4i). Here, we set
H(q,t,p) = (plq) - L(q,4(p,t),t),
where 4 = q(p, t) follows from the equation
P = L,(q, 4, t),
which describes the so-called Legendre transformation. In classical mechanics,
the functions Land H are called Lagrangian and Hamiltonian, respectively.
Moreover, we have
S = action.
The minimum problem (51) corresponds to the principle of least actio',. In
many cases, we have:
L = kinetic energy - potential energy,
H = total energy = kinetic energy + potential energy.
The Hamilton-Jacobi equation plays a fundamental role in the calculus of
variations and in modern control theory. This will be studied in detail in Part
III. Concerning control problems in economics, we have:
S(q, t) = minimal costs of the optimal process,
where q and t are external parameters.
The classical method of solving the Hamilton-Jacobi equation (49) via (SO)
frequently runs into trouble, since singularities may appear. In order to
motivate this in terms of geometrical opti cs, we se t
L = lJ(r,q)c- 1  I + 4 2 ,
where c is the velocity of light and n(t, q) is the index of refraction at the point
(t,q) e A 2 . The solutions q = q(t) of(SI) are light rays in the (t,q)-plane. Note
that t and q are space variables. In this special case, the minimum problem
(51) represents Fermat's principle of least time and we have:
S = minimal value of (S 1) = running time of light.
We expect that S loses its smoothness if light rays bifurcate as in Figure 33.5.
q
(qo. (0)
. ,
Figure 33.5

33.11. A Look at Generalized Viscosity Solutions
949
Our goal is to introduce a notion of generalized solutions of Hamilton-
Jacobi equations (so-called viscosity solutions) which allows general existence
and uniqueness theorems. Observe that viscosity solutions are always con-
tinuous, but not necessarily differentiable. This is reasonable from the point
of view of geometrical optics, since we expect that the running time S depends
continuously on the distance if the index of refraction is sufficiently smooth.
The designation "viscosity solution" is motivated by the follo\\'ing important
fact Instead of the Hamilton-Jacobi equation (49), we consider the regu-
larized parabolic equation
5, - "M + H(q, I, S4) = 0, (52)
where '1 is a small positive number called artificial viscosit}'. As we will show
in Section 70.3, the Navier-Stokes equations for viscous nuids are of the form
(52), where " denotes the viscosity. In fluid dynamics, the limiting process
" ..... 0 corresponds to a passage from viscous fluids to inviscid fluids. This
motivates the following basic idea of our approach:
(a) For each small" ..... 0. we determine a solution S(,,) of (52).
(b) We consider the limiting process SC')  S as " -. o.
(c) S is a viscosity solution of (52) for '1 = o.
This procedure makes sense if we are able to show the following:
(i) The notion of viscosity solution can be introduced independently of the
approximation process above.
(ii) There exist uniqueness theorems for viscosity solutions.
(iii) Classical solutions are viscosity solutions.
(iv) Conversely, C J -viscosity solutions are classical solutions.
This method is called parabolic regularization.
If the Hamiltonian H is independent of time t, we can also consider the
stationar}' Hamilton-Jacobi equation
H(q, S4) = o.
In this case, we use the method of elliptic regularization
- ,,115 + H(q, Sq) = 0, (54)
which leads to viscosity solutions of (53).
In what follo\\'5 we use the following notation. Let M be a subset of IRK. We
set:
(53)
C6'(M)+ = {r4 E Ct(JW): u  0 on M}.
C(M) = {14: M  R, continuous},
Cb(M) = {u: M .-. R. continuous and bounded},
C"b(M) = {14: M  R, uniformly continuous and bounded.}.

950
33. Second-Order Evolution Equations and the Galerkin Method
In the spaces C(M), Cb(M), and C"b(M), we introduce the norm
"ull = sup lu(x)l.
XEM
33.11 b. The Basic Definition
Instead of equation (49), we consider the more general equation
f'(x, S, S') = 0 on G,
(55)
where G is an open subset of IRK. We are looking for a function S = S(x) from
G into R, where S' = (D. S,. . . ,DKS) denotes the tupel of the first partial
derivatives. The Hamilton-Jacobi equation (49) corresponds to (55) with
x = (q,t), K = N + I, and S' = (8 q ,S,). The stationary Hamilton-Jacobi
equation (53) corresponds to (55) with x = q, K = N, and S' = Sq.
Defini.ion 33.21. Let cp E CO(IR N )+ and C E R. The viscosity derivative of the
function S: G  R at the point x with respect to cp and c is defined through
, c - S(x) ,
S."c(x) = rp(x) rp (x),
where we assume that cp(x) # o.
Remark 33.22 (Motivation). Let S E C.(G), where G is open. Suppose that x
is a minimal point of
min cp(y)(S(y) - c) = cp(x)(S(x) - c) < 0,
YEG
(56)
or a maximal point of
max cp(y)(S(y) - c) = cp(x)(S(.t) - c) > o.
)'E G
(57)
Then the viscosity derivative is equal to the classical derivative, i.e.,
S.c(x) = S'(x).
This follows from (cp(S - c))' = 0 at ., and hence
(S(x) - c)cp'(x) + cp(x)S'(x) = O.
Defini.ion 33.23. A viscosity solution of equation (55) is a continuous function
S: G ..... R such that for every cp E C(G)+ and C E R the following hold:
(i) If the solution set of (56) is not empty, then there exists a minimal point
x of (56) such that
F(x, S(x), S.c(x)) > o.

33.11. A Look al Generalized Viscosity Solulions
951
(ii) If the solution set of (57) is not empty, then there exists a maximal point
x of (57) such that
F(x, S(x), S.c(x)) < o.
33.11c. Properties of Viscosity Solutions
According to Remark 33.22, each classical C1.solution of (55) on an open set
G is also a viscosity solution. Conversely, one can prove the following.
Proposition 33.24 (Consistency). Let F: G x IRK + I --+ R he continuous, Vtthere G
is an open sahset of IRK, K > 1. If s: G -+ R is a viscosity solution of the original
equation (55). and if the classical derivative S'(x) exists, then the function S
satisfies (55) at the point x.
The methods of parabolic and elliptic regularization described above are
based on the following result.
Proposition 33.25 (Stability). Let F i : G x nl: + I --+ rR be continuous for all kEN,
",here G is an open suhset of IRK, K > I. For each k, let Sit e C(G) be a I,iscosity
solution of the equation
F,,(x, Si' S) = 0 on G.
As k ...... 00, let
Fie -+ F in C(G x R K + 1 ),
S, --+ S in C(G).
Then S is a l,jscosity solution of the equation
(58)
(59)
F(x,S,S') = 0 on G.
The convergence in (58) and (59) means uniform convergence on each
compact subset of G x R K + 1 and G, respectively.
33.11d. The Initial Value Problem
We consider the following initial value problem:
S, + H(Sq) = 0 on R N x ]0, oc.(,
S(q, 0) = So(q) on R N .
(6Oa)
(60b)
Theorem 33.F (Crandall and Lions (1983)). Let HeC(R N ), N > 1. For
each given So E Cub(R N ), there is a unique function S which has the folloVt'ing

952
33. Second-Order Evolu(ion Equa(ions and the Galerkin Method
properties:
(i) S e C(R N x [0, oo[) n CMb(R'" x [0, T]) for all T > O.
(ii) S is a viscosil.V solution of eqrlatiolJ (6Oa), and the initial condition (60b) ;s
satisfied.
(iii) lim sup IS(q, t) - So(q) I = o.
, +0 qc R'"
Morever.. r we set Y>(t)So(q) = S(q, t), ",en {((t)} is a lJolJexpansive semi-
group on Cub(IRH).
The proof uses the method of parabolic regularization.
33.11 e. The Stationary Case
We now study the follo\\'ing equation
S + 1/(5 4 ) = f(q) on R"..
(61)
Proposition 33.26. Let H e C(R".} alullet Ie CMb(R N ). Then equation (61) has a
unique I,;scosity soilltion 5 e Cb(R''').
The proof uses the method of elliptic regularization.
EXAfPLE 33.27. The equation
S + IS 4 1 = 1 on R (62)
has the following infinite family of Lipschitz continuous, bounded solutions:
{ I - Ce q if q  0,
S(q) = I - Ce- q if q  O.
where C > 0 is an arbitrary constant. The uniquely determined viscosit}'
solutio,! of(62) corresponds to C = o. If C > 0, then we obtain solutions \vhich
satisfy equation (62) except at the point q = o.
The regularized equation
- "Sft + S + IS 4 1 = 1 on R
has the classical solution
S(q) = 1 + e- 2il12 ", a = 1 + J I + 4'1,
which goes to the viscosity solution S = I of (62) as " -+ + o.
The proofs to the results mentioned above and more general theorems
can be found in Crandall and Lions (1983) and Lions (1983, L). We also
recommend the survey article by Lions (1983a).

Problems
953
PROBI.MS
33.1. Proof of Len'lna 33.1.
Solution: Noting A(s)(O) = 0 as well as the monotonicity and coercivcness of
At we obtain that
1 (A(s)u;(s). u(s» ds > 0 for all t E [0. T]. (63)
and
f: (A(s)u(s).u(s»ds  "llIu;:' - "2 T. (64)
By (32.79) and the strong monotonicity or L. there is a c > 0 such that
2 L (Lu.(s ,,;(s» = (Lun(t). u,,(t»
 c Uu.(r)" 2 for all I E [0. T]. (65)
Moreover, since b E L 2 (O, T; 1 we obtain that
I (b(s)lu(s))ds  1 I b(s) I! u;(s) I ds
I f '
 2 0 Ib(s)1 2 + lu(s)12 ds
 const (I + L lu(s)l: dS) for all I E [0. T].
Since u e W"I(O, T; II: H) and u(O) = 0, integration by pans yields thc ke}'
estimate:
!IU;(t)l2 - L (u:(s).u(s)ds
- I - (Au.u) - (Lun'u> + (blu;)ds
;5; const (I + I lu(s)12 dS) for all t E [0. T]. (66)
In this connection, \\'C usc the Galerkin equation (3) and (63)-(65).
(I) Using (66) and the Gron",.allll'mma from Section 3.5. we obtain that
I u(I)1 < const for all I e [0. 11 and all n.
(II) From (66) with I = T and (64), (65), we get
o < f: - (Au;.u;) - (Lu".u> + (blu;)ds
< - c.llu;IJ + "1 T + const (1 + f: lu;(sW dS)
s -cll1u;U + consl.

954
33. Sccond-Order Evolution Equations and the Galerkin Method
where c 1 > O. Hence
I, u II % :$; const
for all n.
(III) Finally, we obtain from (63)-(66) that
o  f - < Lu.(s). u;(s) > + (b(s)lu;(s)) d.s
< - C lIu,,(1)1I 1 + cons
and hence
II u.( t) 1/ s const
for aU t e [0, T] and all n.
33.2. Proof of Lemma 33.2.
Solution: Let u' E WJ'I (0, T; II: H) and u(O) ::: O. According to Step S of the
proof of Theorem 23.A. for each n E N, there exists a polynomial P.: [0, T] ...
Jt;. with coefficients in It;. such that
PIt  u' in WI" (0, T; I'. H)
and p,,(O) -. u'(O) in H as n  00. That means
lip. - u'!) x + Ilp - uNII..._ .... 0
as n-+oo
as n -t 00.
We set
qR(t)::o J PR(S)d.s for all t e [0, T].
Hence q,,(O) = 0 and q = P... The continuity of the embedding W". (0. T; V. H) c
C([O, T], H) implies that
P. ... u' in C([O, T], H)
as n.... 00.
Finally, it follo\\'s from the !-folder inequality that, for allt e [0, T),
flqR(t) - u(t)... S J (q; - u')ds t
$ const IIq - u' x ..... 0
and hence q. -+ u in C([O, T], V) as n ..... 00.
33.3.. A semilinear WQt'e equation. We consider the folloy,'ing initial value problem:
as n.... CO,
U lt - &, + lul,-1 u = f(x,t) on G x ]0, T[,
u(x,O) ::I uo(x)
and
u,(x,O) == vo(.) on G
(67a)
(67b)
along with the boundary condition
u(x. t) = 0 on iJG x [0, T].
Let G be a bounded region in R N , and let 0 < T < co, 2 < p < 00, N  1. We
.
arc given
Uo e Y, Vo e L 2 (G), J-e L 2 (G x ]0, T[),
where Y - J('l(G) ('a LJ'CG). We equip Y with the norm max{lIull..1, DullJ'}.

Problcms
955
Show that problem (67) has a solution
u e L(O, T; Y).
u, e Loo(O, T; L 2 (G)).
(68)
Moreover, show that it follows from (67a) and (68) that
u e C([O, T], L 2 (G)).
u, e C( [0, T]; Y.).
In this connection use Problem 23.13. Thus. the initial condition (67b) makes
sense. Finally, show that the solution is unique if p - 2 > 0 is sufficiently small.
The solution is to be understood in the generalized sense as in Section 33.5.
i.e.. all the derivatives arc to be understood in the sense of distributions.
Hint: Use a Galerkin method. See Lions (1969, M). Section 1.3. Observe that
Y. = Wl(G)* + Lp(G). (see Problem 23.14).
33.4. Conservation laM's and shocks. In connection with Section 33.8, study Smoller
(1983, M) and Lax (1984. S).
33.5. Semilinear 'ave equations. In connection with Section 33.10, study the funda-
mental papers b)' John (1985), (1987), (1988), Klainerman (1985a). (1985) and
the monograph by Christodoulou and Klainerman (1990
33.6. Semilinear hyperbolic systems in two variables and the Colombeau algebra of
generalized distributions. Study Oberguggenberger (1987). In this paper, semi-
linear first-order hyperbolic systems in two variables (i.e., one space variable)
are considered. whose nonlinearity satisfies a global Lipschitz condition. It is
shown that such systems admit unique global generalized solutions in the
so-caJled CoJombeau algebra (1R2) of distributions. In particular, this provides
unique generalized solutions for arbitral)' distributions as initial data. The
generalized solutions arc limits of smooth approximate solutions. Note that the
Colombeau algebra contains the set of distributions (R.ti). and (RN) is a
subalgebra Of(IRN) (see Colombcau (1984, M), (1985, M)
Further interesting results on semilinear hyperbolic equations in two vari-
ables can be found in Rauch and Reed (1980), (1981), (1982), (1988).
33.7. Explicit solutions of evolution equations. Cf: Problem 30.7.
33.8. Generalized solutions of the Hamilton-Jacobi equations. In connection with
Section 33.11, study Lions (1983, L) and Crandall and Lions (1983
33.9.$ Blowing-up effects for nonlinear strings. We consider the nonlinear string
equation
u" = g(u.x)u.x x '
o s x S Jr, t > 0,
u(x 0) = uo(x). ",(x, 0) = 0, 0 S x S Jr,
u(O, I) = u(1t t) = O. t  0,
(69)
with
9 ;;I c 2 (1 + auf, uo(.) ;II; £ sin x,
where c, a, I; > 0 and a£ is small. Here, u - u(., t) describes the shape of the
string at time I.
Reduce equation (69) to a first-order system and use Example 33.14 in order

956
33. Second-Order E\'olution Equations and the Galerkin tcthod
to show that the classical solution of (69) breaks down after the time
Te,il  4/c at.
This formula is asymptotically true for ae  0,
Hint: cr. Lax (1964). This paper contains general results for quasi-linear
hyperbolic first-order systems in two variables.
References to the Literature
Classical existence proofs for quasi.linear hyperbolic equations of second order:
Schauder (1935), Leray, (1952, M) (systems of equations).
Introduction to nonlinear hyperbolic equations: Courant and HiJben (1953. M).
Vol.  Smoller (1983, M), Majda (1984, L).
Monographs containing important results on nonlinear hyperboJic equations:
Leray (1952), Lichnerowicz (1967). Lions (1969), Strauss (1969), Hawking and Ellis
(1973), Gajewski, Groger, and Zacharias (1974), Barbu (1976). Jeffrey (1976). Rccd
(1976), Haraux (1981), Choquet-Bruhat (1982a), Marsden and Hughes (1983). PaZ}'
(1983) (thoor)' of semigroups), John (1985) (collected papers). Christodoulou and
Klainerman (1990).
Nonlinear wave equations in mathematical physics: Courant and Hilbert (1953. M).
Vol. 2. Jeffrey and Taniuti (1964, M) and Lichnerowicz (1967. M) (magnetohydro-
dynamics). \Vhitham (1974.. M) (nonlinear waves), Kato (1975, S) (many important
examples), (1986, S Reed (1976, L) (quantum theory), Marsden and Hughes (1983. M)
and John (1985) (elasticity), Majda (1984, L) (fluid dynamics).
Einstein equations of general relativity and the Einstein- Yang- Mills equations:
Hawking and Ellis (1973, M), Choquet u Bruhat and Yorke (1980, S), Choquct-Bruhat
and Christodoulou (1981  (1982), Arms, Marsden, and MoncrietT (1982), Eardley and
MoncriefT (1982), Klainerman (1987, S), Christodoulou and Klainerman (1990. M)
(cf. also the References to the Literature for Chapter 76).
Gas d)'namics: Smoller (1983, M) (cf. also the References to the Literature for
Chapter 86).
Vlasov-Max\\'ell equations in plasma physics: Wollman (1984).
Boltzmann equation in molecular gas kinetics: Albeverio (1986, M) (cf. also the
References to the Literature for Chapter 86).
Mathematical viscoelasticity: Renardy, Hrusa, and Nohel (1987, M) (fundamental
monograph Nohel. Rogers, and Tzavaras (1988
Solitons: cr. the References to the Literature for Chapter JO.
Linear symmetric hyperbolic S)'stcms: Friedrichs (1954) (classical paper), (1980)
(collected papcrs Courant and Hilbert (1953. M Vol. 2, John (1982, M
Quasi-linear symmetric h)'perbolic systems: Kato (1975, S), (1975a), (1986, S),
H ughcs. Kato, and Marsden (1977), Majda (1984, L) (cf. also the References to the
Literat urc for Chapter 83).
Consen'ation 18\\'s: Lax (1973, S), (1984, S), Bardos (1982, L), Smoller (1983. M),
Majda (1984. L),
The interaction of nonlinear hyperbolic waves: Glimm (1988, S).
Blow-up of the solutions for nonlinear wave equations: Lax (1964), John (1916),
(1985) (collected papers). (1987), Klainerman (1983. S) (recommended as an introduc-
tion), Shatah (1982), K lainerman and Ponce (1983), John and K lainerman (1 984
Klainerman (1985). (1985a), Christodoulou (1986), Christodoulou and K laincrman
(1990, M).
Almost global existence of clastic waves: John (1988),

References to the Uterature
957
Self.similar blow-up: Bcbcmcs and Eberly (1988).
Resealing algorithm for the numerical calculation of blow-up: Berger and Kohn
( 1988).
Generalized solutions for scmilinear hyperbolic systems in two variables and
the propagation of singularitics: Rauch and Reed (1980 (1981 (1982), (1988),
Obcrguggenberger (1986). (1987). (1988) (Colombeau algebra of generalized distribu-
tions; the initial value data are distributions).
Colombcau algebra of generalized distributions: Colombeau (1984. M (1985, M).
Propagation of singularities and paraditrerential operators: Bony (1981), (1984, S),
Runst (1985). Lebeau (1986).
Generalized solutions (viscosity solutions) of Hamilton Jacobi equations: Lions
(1983. L). (1983a. S). Crandall and Lions ( 1983), (1985).
Periodic solutions for the nonlinear string equation: Rabinowitl (1978 (1984a
Brczis and Nirenberg (1978a). Brezis, Coron, and Nirenberg (1980).
Blow-up for the nonlinear string equation: Lax (1964), Klainerman and Majda
(1980).
Conferences on nonlinear hyperbolic partial differential equations: Carasso (1987,
P). Ballman (1988, P).
(Cf. also the References to the Literature for Chapter 30).

GENERAL THEORY OF
DISCRETIZATION METHODS
The interplay between generality and individuality, deduction and construc-
tion, logic and imagination-this is the profound essence of live mathematics.
Anyone or another of these aspects can be at the center of a given achievement.
In a far-reaching de\'elopment all of them will be involved. Generally speaking.
such a development will stan from the "concrete ground," then discard ballast
b)' abstraction and rise to the lofty layers of thin air where navigations and
obsen'ations are easy: after this flight comes the crucial test of landing and
reaching specific goals in the newly surveyed low plains of individual "rcalit),."
In brief, the flight into abstract generality must stan from and return to the
concrete and the specific.
Richard Courant (1888-1972)
We want to explain some basic ideas of general discretization methods.
Suppose that we are given the operator equation
(E)
Au= b
,
ue X,
which may correspond to a differential or integral equation. In order to solve
(E) by means of an approximation method, we replace the operator A: X -+ y
by an appropriate approximation All: X n -+ , where X" and Y n are finite-
dimensional spaces, and we replace the right-hand side bEY by b. e . This
y,'ay we obtain the approximate problems
(E,,)
A"u" = b. t
U" e XII'
" = 1, 2, . . . ,
which correspond to linear or nonlinear systems of equations with finitely
many unknowns. Such problems can be solved on computers. For example,
equations of type (Eft) arise in connection with the Galerkin method and the
difference method. Note that we do not assume that X" £; X and  !;; y: For
example, this is important for describing difference methods by (Eft)' In this
959

960
General Theory of Discretization Methods
case, X and Y are spaces of functions defined on the region G, whereas XII and
 are spaces of functions defined on the set of grid points.
For numerical mathematics it is of great importance to answer the following
two questions.
PRoBLat I. Which conditions must be satisfied by X, Y, XII' Y II , A, All in
order to obtain the following? If the original equation (E) has a unique
solution, then the approximate problems (Ell) have unique solutions and the
approximation method converges.
This problem can be formulated briefly like this:
Wi,e,. is it possible to conr.,'erl a nonconstructive existence proo.f into a
constructive e"..;istence proof?
PROBLEM 2. Which conditions must be satisfied by X, 1': X,., , A, Aft in
order to obtain the following? If the approximate problems (Ell) are uniquely
solvable, then the approximation method converges to a solution of the
original problem (E).
We shall give a final answer to Problems 1 and 2. Roughly speaking. we
shall prove the following main result of numerical functional analysis.
If the approximation method is consistent and stable, then the following three
conditions are equivalent.
(Cl) Solvability. The original problem (E) ha.. a solution, i.e., we kno\\' an
abslract exiSlelJce proof for (E).
(C2) Unique approximation-solvability. The approxilnate problems (E,.) have
unique soilltions and these solutions converge strongl}J to the unique solu-
lion of the original problem (E), i.e., we obtain a constructive ex;sre,.ce
proof
(C3) A-properness. The operator A: X -. Y is A-proper.
This underlines the fundamental importance of A-proper operators for the
general theory of approximation methods. This class of operators was intro-
duced and studied in detail by Petryshyn (1967) (1968fT). An extensive survey
of the general theory of A-proper operators and its applications can be found
in Petryshyn (1975).
For example, the following operators A are A-proper:
(i) A = B + C, where B is uniformly monotone and continuous, and C is
compact.
(ii) A = + I + K + C, where K is k-contractive and C is compact.
(Hi) A = B + C, where B is strongly stable (e.g., strongly monotone) and
continuous, and C is compact.

General Theory or Discretization Methods
961
Therefore, the theory of A-proper operators unifies the following funda-
mental results under a constructive aspect:
The fixed-point tlaeorem of Banach (A = I - K).
1.he fixed-point theorem of Schauder (A = I - C).
The existence theorems on monotone and pselldomonotone operator eqrlations.
The next two results can be used to prove the A-properness of perturbed
A-proper operators:
If the operator A is A-proper and if C is compact, then A + C is also
A-proper.
If A is A-proper on a normed space over IK, then ,iA is also A-proper for
each nonzero A E IK.
Our plan is the following. In Chapter 34 we consider so-called inner
approximation schemes which correspond to the Galerkin method.
In Chapter 3S we consider external approximation schemes which corre-
spond to the difference method.
In Chapter 36 we introduce a mapping degree for A-proper operators.

CHAPTER 34
Inner Approximation Schemes, A-Proper
Operators, and the Galerkin Method
For what type of a linear or nonlinear mapping A is it possible to construct a
solution u of the equation
Au = b
as a strong limit of solutions u. of the simpler finite-dimensional equations
A.u" = Q"b?
In a series of papers the author studied this problem. and the notion which
evolved from these investigations is that of an A-proper mapping. It turned
out that the A-properness of A is not only intimately connected with the
approximation-solvabilit), of the equation Au = b but, in view of the fact that
the c)ass of A-proper mappings is quite extensive. the theory of A-proper
mappings extends and unifies earlier results concerning Galerkin type methods
for linear and nonlinear operator equations with the more reccnt results in the
thcol)' of strongly monotone and accretive operators, operators of type (S),.
P,-compact, ball-condensing and other mappings.
w. V. Petryshyn (1975)
34.1. Inner Approximation Schemes
Along with the operator equation
Au = b,
we consider the approximate equations
ue X,
(I)
A"u" = Q"b,
u" eX",
n = I, ...,
(2)
which correspond to the following approximation scheme:
963

964 34. Inner Approximation Schemes. A.Proper Opcrato and the Galerkin Method
X A) Y
R. H  1 Q.,
A.. = Q..AE...
(3)
A. v
X" . '..
The operator R,,: X -. X" is called a restriction operator, and E,,: XII  X is
called an e.,'(tension operator. An important role will be played by the so-called
compatibility condition:
lim IIEIIR"u - ullx = 0
for all 14 e X.
(4)
"  c:t>
EXA1tIPLE 34.1 (Galerkin Method in H-Spaces). Let (e.) be a complete ortho-
normal system in the separable infinite-dimensional H-space X. We set
X" = span {e 1 ,..., e,,}, n = I, 2, ... .
Let P,.: X -. X" be the orthogonal projection operator from X onto X"' i.e.,
"
P"u = L (e.lu)e.
1=1
for all U E X.
Moreover, let
E,,: X,.  X
be the embedding operator corresponding to X"  x. In order to obtain (3
we set
Y = X.
y. = X..,
R. = Q. = PIt.
It is easy to check that this approximation scheme is an admissible inner
approximation scheme in the sense of Definition 34.2 below. In this connec-
tion. note that IIP..II = I for all n and that P"u -+ u as n -+ CX) for aU u e X.
Let the operator A: X  X be given and let
A.. :: PIIAE...
Then the approximate problems (2) correspond to a projection method
(Galerkin method). For n = 1,2,..., equation (2) is equivalent to the following
Galerkin equations:
(Au..lej) = (bl ej),
u" eX",
j = 1, ..., II.
Defmition 34.2. The tupel {X, X., y, Y,.,R"tE"tQ..}lIcN represented in (3) is
called an adlnissible inner approximation scheme iff the following hold.
(i) X and Yare infinite-dimensional normed spaces over K = R, C.
(ii) X" and t:. are normed spaces over I< with dim X. = dim f" < 00 for all n.
(iii) For all II, the operators E,,: XII ..... X and Q,,: Y -+ t:. are linear and con-
tinuous with
sup.. II E.II < \t) and sup..IIQ,,1I < 00.
(iv) The compatibility condition (4) is satisfied.

34.2. The Main Theorem on Stable Discretization Methods
965
In contrast to Example 34.1, we do not postulate that X" c: X and Y.. c Y,
i.e., we allow the original problem (I) and the approximate problems (2) to
live in completely different spaces. However, we postulate A.. = Q"AE", i.e.,
the diagram (3) is commutative. In the next chapter, this condition will drop
out by using external approximation schemes.
In connection with diagram (3), there are two different possibilities of
defining the convergence of the approximation method, namely, we can use
either
lim IIE..u.. - ull x = 0,
..-x
or
lim lIu.. - R..ull x = o.
"
..-x
In this chapter (resp. in the next chapter), we will use the first (resp. second)
possibility.
34.2. The Main Theorem on Stable Discretization
Methods with Inner Approximation Schemes
Our goal is to investigate the connection between the following conditions
(C I) through (C3).
(C I ) Solvability. For each bEY, the original equation Au = b, u EX, has a
solution.
(C2) Unique approximation-solvability. The original equation is uniquely
approximation-solvable, i.e., for each bEY, the following hold:
(i) The equation Au = b, u E X, has a unique solution.
(ii) For each n > no, the approximate equation A..u.. = Q..b, u.. EX",
has a unique solution.
(iii) The sequence (u..) converges to the solution U of the equation Au = b
in the sense of lim.._ II E..u.. - ull x = o.
(C3) A-properness. The operator A: X -+ Y is A-proper with respect to the
approximation scheme (3). That is, by definition, the following holds. Let
(n') be any subsequence of the sequence of natural numbers. If (u...) is a
sequence with U,,' E X". for all n' and if
(i) lim" _ II A". U,,' - Q...b II r = 0 for fixed bEY, and
"
(ii) sup".lIu".lI x < 00,
"
then there exists a subsequence (u"..) such that
lim II E.... U.." - u II x = 0
II-CC
and Au = b.
Examples for A-proper operators will be given in Sections 34.4 and 34.5.
The designation uA-proper" stands for uapproximation-proper." Note that

966 34. Inner Approximation Schemcs. A-Proper Operators. and the Galerkin Method
A-proper operators represent a modification of proper continuous operators.
To show this let A: X -+ Y be proper. This means that the preimages A-1(K)
of compact sets K are again compact. Thus, it follows from
Au", -+ b
as n -+ 00
that there is a subsequence (u",,) such that
14,," -+ U
as n --. 00.
If, in addition, the operator A is continuous, then Au = b.
The following theorem is the main result of this chapter.
Theorem 34.A (Petryshyn (1970)). The three conditions (CI) through (C3) are
nU4tuaily equivalent in the case where the following hold.
(H I) Approximation scheme. The diagram (3) represents an admissible inner
approximation scheme. All the operators All: XII -+ Y" are continuous.
(H2) Consistency. f'or all u E X,
lim IIQ"Au - A"Rllulir = o. (5)
"
II-:L
(H3) Stability. There ;s an no such that
II A" u - A" v II r > a ( II u - v II x ),
" "
(6)
.for all 14, v E XII and all n > no. Here, the given function a: IR+ -+ R. ;s
continuous and strictly monotone increasing with a(O) = 0 and a(t) -+ +00
as t -+ +00 (/:;g. 34.1).
Roughly speaking, this theorem tells us the following:
If the approximation method is consistent and stable, then the original equa-
tion Au = b, U E X, is uniquely approximation-solvable iff the operator A is
A-proper.
Before proving Theorem 34.A, we consider some variants of this theorem.
Corollary 34.3 (Sufficient Condition for Consistency). If the operator A: X --. y
is continuous, then (H I) implies (H2).
.
Figure 34.1

34.2. The Main Theorem on Stable Discretization Methods
967
Corollary 34.4 (Lack of Consistency Suppose that the assumptions (HI) and
(H3) hold and suppose tllat A: X  Y is A-proper. Then:
(a) For eacl. beY, the equation Au = b, u e X, has a solution.
(b) For each bEY and each n  no, the approximate equation A"u" = Q"b,
u.. E X., lIas a unique solrlt;on.
(c) The sequence (u lI ) has a subseqllence (u..-) ",hicl, converges to a solution u of
the original eqrlalion All = b, U e X, in lhe sense of
lim II E..,u". - uN % = o.
" -00
(d) If. for fixed bEY, tile equation Au = b, U E X, has a unique solutio then
the elltire sequence (u.) contJ'erges to u.. i.e.,
lim II E..u" - u II x = o.
"  a:>
Corollary 34.5 (Compensation for the Lack of Stability by a priori Estimates).
Suppose that:
(i) The operator A: X --. Y is A-proper '\lith respect to the admissible inlier
appro..imation scheme (3).
(ii) For fixed bEY alJd all n  no, the approximate equation Anu" = Q.b,
u"e X., lias a uIJique solutioll, and we have the a priori estimate sup"lIullll. t .. <
ex). Then:
(a) There exists a subsequence (u".) such that
lim II E".u II - - 1111 x = 0
and
Au = b.
"  CI()
(b) If the eqllatiolJ Au = b, u e X, has a Ilniqlle Solulion, then
lim IIEnu. - ullx = O.
.
Corollary 34.6 (Compact Perturbations). If the operator A: X  Y is A -proper
with respect 10 the admissible i,mer approximation scheme (3), and if C: X -. Y
is compact, then A + C: X -+ Y is also A-proper.
If A: X -+ Y is A-proper, then so is ).A for all nonzero ,i e IK, wltere X and
Y are normed spaces over K.
Remark 34.7. Let the above assumptions (HI) through (H3) be satisfied. Then
there are two different possibilities of using Theorem 34.A.
(i) Abstract existence theorems imp')' the unique approxinwtion-solvabilit}7.
Suppose that we know that the equation Au = b, u EX, has a solution,
i.e., condition (CI) holds. Then Theorem 34.A says that this equation
is uniquely approximation-solvable and the operator A: X  Y is A-
proper.
By Corollary 34.6, the compact perturbation A + C: X -+ Y is also
A-proper.

968 34. Inner Approximation Schemes, A-Proper Operators, and the Galerkin Method
(ii) A-properness implies the unique approximation-solvability. Conversely,
suppose that we can prove the A-properness of A: X --+ Y by means of a
direct argument. Then Theorem 34.A tells us that the equation Au = b,
u E X, is uniquely approximation-solvable.
34.3. Proof of the Main Theorem
We prove Theorem 34.A. The main idea of proof is to use the theorem on the
invariance of the domain from Section 16.4, which follows from the antipodal
theorem.
Step I: (C3) implies (C2).
(I) We show that, for fixed bEY and each n > no, the approximate equation
A"u" = Q"h, U" E X"' has a unique solution. To this end, we will use the
invariance of domain theorem.
(I-I) The set A,,(X,,) is open. Indeed, the operator A,,: X" --+ Y" is injective,
by the stability condition (H3). The invariance of domain theorem
(Theorem 16.C) tells us that A,,(X,,) is open.
(1-2) The set A,,(X,,) is closed. To prove this let A"u,  z as k --+ 00. Thus (A"u,)
is a Cauchy sequencc. By (H3), (u i ) is also a Cauchy sequence in X".
Hence u, --+ u as k ..... 00. Since A" is continuous, we get A"u = z, i.e.,
Z E A,,(X,,).
(1-3) Since the nonempty set A,,(X,,) is open and closed in Y", we obtain
A,,(X II) = Y" (cf. A I (13f».
(II) We show that, for fixed bEY, the equation Au = h, u E X, has at most
one solution. To this end, let Au = Av. By consistency (H2) and stability
( H 3).
a(IIR"u - R"vll) < IIA"R"u - A"R"vll
< flA"R"u - Q"AuU + IIQ"Av - A"R"vll ..... 0 as n..... 00.
Hence II R"u - R"vll -. 0 as n --+ 00. Since sup" II E"II < 00,
IIE"R"u - E"R"vll x -.0 as n  00.
Now, the compatibility condition (4) yields u = v.
(III) We prove that II E"u" - 1411x ..... 0 as n -. 00 and Au = b. For fixed hEY
and n > no, we solve the equation A"u" = Qllh, u" E X"' according to (I).
From
A,,(O) = Q"AE,,(O) = Q"A(O)
and stability (H3) it follows that
a(IIu"lI) < IIA"u" - A,,(O)II  UQ"II(IIbil + IIA(O)II).
Since sup"UQ,,1I < 00, we obtain sup"a(lIu"II) < 00 and hence the a priori

34.4. Inner Approximation Schemes in H-Spaces
969
estimate:
sup"lIu.1I < :x).
Obviously, [JA"u.. - Q..bll --.0 as II -. 00. The operator A is A-proper.
Thus, there is a subsequence (u,,-) such that
II E...u,,' - ull x --. 0 as n -. 00. (7)
and Au = b.
The same argument shows that each subsequence of(E"u,,) has, in turn.
a subsequence (EII,u".) satisfying (7). By the unique solvability of Au = b
the limit u in (7) is the same for all subsequences. Therefore, it follows
from the convergence principle (Proposition 1 O.13( 1) that relation (7)
holds for the entire sequence (E"u lI ).
Step 2: (C2) implies (Cl). This is obvious.
Step 3: (CI) implies (C3).
For given b e  we solve the equation Au = b, u e X. To prove that
A: X -+ Y is A-proper, assume that sup".lIu..1I < 00 and
lim IIA..u". - Q".bll = o.
.
For brevity we will write u" instead of u.-. By consistency (H2) and stability
(H3),
a(III'" - R"ull) S IIA"u" - A"R.u[)
S IIA"u" - Q"bll + IIQ.Au - A"R.ul1  0 as n -+ CX).
Hence II u" - R"I'II  0 as n -+ rot Since SUp" II E"I' < 00,
II E"u.. - E"R..ull  0 as n  00.
The compatibility condition (4) yields
II E"R"u - u II  0
as n  00.
Hence HE.II" - ull -. 0 as n  00, i.e., A is A-proper.
This finishes the proof of Theorem 34.A. The proofs for CoroUaries 34.3
through 34.6 will be given in the problems section to this chapter.
34.4. Inner Approximation Schemes in H-Spaces
and the Main Theorem on Strongly
Stable Operators
We consider the operator equation
Au = b,
ue X,
(8)

970 34. Inner Approximation Schemes, A- Proper Operator and the Galerkin Method
together with the approximate equations
PIt Au" = P"b,
14" EX",
n = I, 2, . . . ,
(9)
corresponding to the following approximation scheme:
X A X
.
p. H E 1 p.. A" = P"AE". ( 10)
.
X" A. . X"
We make the following assumptions.
(A 1) X is a separable H-space over II( = R, C with dim X = 00, and (e , ) is a
complete orthonormal system in X. Let
X" = span {e l' · · · ,e" },
and let PIt: X  X" be the orthogonal projection operator from X onto
X II' i.e.,
"
P"u =  (e;l u}e;.
i= 1
Let E,,: X" -... X be the embedding operator corresponding to X" £; X.
(A2) The operator A: X  X is continuous and strongly stable, i.e., there is
an a > 0 such that
I(Au - AI'lu - v)1 > a lIu - vll 2
for all 14, v EX.
(A3) Let A = B + L, where B: X  X is continuous and strongly monotone,
and L: X  X is Lipschitz continuous (e.g., L = 0). More precisely, we
assume that there are numbers c, d with 0  d < c such that, for all
14, v E X,
Rc(Bu - Bvlu - v) > cllu - vll 2 t
and
II Lu - Lv"  d II 14 - v II.
(A4) Let A = + I + K, where K: X -... X is k-contractive.
Obviously, (A3) and (A4) are special cases of (A2). For example, consider
the operator A in (A3). Then, for all u, v E X,
I(Au - Avlu - v)1  Re(Au - Avlu - v)
= Re(Bu - Bvlu - v) + Re(LII - Lvlu - v)
 (c - d)lIu - vll 2 ,
i.e., A satisfies (A2). In case (A4) we obtain
I(Au - Avlu - v)1 = 1(14 - vlu - v) + (Ku - Kvlu - v)1
> (I - k)" u - V 11 2 for all u, v E X,

34.4. Inner Approximation Schemes in H-Spaces
971
since IIKu - Kvll < kllu - vII for all u, VE X with 0 < k < 1, i.e., A satisfies
(A2).
By Example 34.1, the diagram (10) represents an admissible inner approxi-
mation scheme. For n = 1,2, ..., the approximate equation (9) is equivalent
to the following Galerkin equations:
(Au"le j ) = (ble j ),
u"eX"..
j = I.. . . . .. n.
Theorem 34.8. Assume (AI), (A2). Then.. for each be X.. the equation Au = b,
u E X, ;s uniquely approximation-soll'able.
The operator A is A-proper K'ith respect to the approximation scheme (10).
If c: X --. X is compact, then A + c: X --. X is also A-proper K'ith respect to
( 10).
Corollary 34.8. Assume (A I). Let A: X  X be continuous and strongly mono-
tone, and let C: X -+ X be compact. Then for each nonzero A E IK, the operator
A(A + C): X -+ X
is A-proper with respect to (10). In particular, the operator
+ I + K + C: X -+ X
is A-proper with respect to (10) if K: X --. X is k-contraclive and C: X -+ X is
compact (e.g., K = 0 or C = 0).
PROOF. We use Theorem 34.A. We shall prove the A-properness of A by a
direct argument.
(I) Consistency. Since A: X -+ X is continuous, the consistency follows
from Corollary 34.3.
(II) Stability. We want to show that
II P" A u - PIt A I'll > a II u - v II
Indeed, for all u, v E X".. we obtain
a lIu - v 11 2  I(P"U - P"L'I Au - Av)1
= f( u - v I P" Au - P" At') I < II u - villi P" A u - P" A l' II.
forall u,veX".
(III) The operator A: X --. X is A-proper. To show this, let sup"llu,,11 < 00
and
IIP"Au" - P"bll -+ 0
as n --. ,
where U" e X" for all n. Since X is reflexive, there exists a subsequence,
again denoted by (u,,), such that
u"  u in X
as n -+ rxJ.
We have to show that
u" -+ u in X
as n -+ 00
and Au = h.

972 34. Inner Approximation Schemes. A-Proper Opcrato and the Galerkin Method
(III-I) We will show in Problem 34.S tha as n ... 00,
u u
..
implies
u - p. uO
" "
(11 )
and
v -+V
n
implies
PIt v"  v.
(12)
Thus, we obtain that, as n  00,
P"b -+ b,
p.U -. II,
AP"u -+ Au,
P.AP"u ... Au,
and hence
P.(Au,. - AP"u) = (P"Au. - P"b) + (P"b - P"AP"u) -+ b - Au.
(13)
(111-2) From (11) and (13) it follows tha as n -+ 00,
a lIu.. - P"u1l 2 S I(Au.. - APnulu" - P"u)1
= I(Pn(Au" - AP"u)lu n - Pllu)1  O.
Therefore, 14" - P"u  0 as n -. 00 and hence Un - U -.0 as II  00.
Thus, Au = b by the continuity of A.
Theorem 34.8 follows now from Theorem 34.A.
Corollary 34.8 follows from Corollary 34.6. 0
34.5. Inner Approximation Schemes in B-Spaces
We consider the operator equation
Au = b,
I' E X,
(14)
together with the approximate equations
E: AE"u" = E:b,
Un EX.,
n = 1, 2, . . . ,
(15)
corresponding to the following approximation scheme:
X A . X.
It. II E. 1 E:. A" = E: AE". (16)
X" A. ) X.
"
We make the following assumptions.
(AI) X is a real separable reflexive B-space with dim X = 00. Let (X,,) be a

34.5 Inner Approximation Schemes in B..Spaces
973
Galerkin scheme in X with
x,. = span {\\'1,.,. . ., K',.,,.},
n = 1, 2, . . . .
Let E,.: X,. --. X be the embedding operator corresponding to X,. c x.
By Problem 34.6, for each u EX, there exists at least an element
R,.u E X,. such that
lIu - R,.ullx = dist(u, X,.).
This way we construct the operator R,.: X -+ X,., by choosing a fixed
solution R"u.
For n = I, 2. .... the approximate equation (15) is equivalent to the
Galcrkin equations:
< Au". "-j,.) = < b, Kj,,), U,. E X n' j = I, . . . , n'.
We will show below that the diagram (16) represents an admissible
inner approximation scheme.
(A2) The operator A: X -+ X. is uniformly monotone and continuous.
Proposition 34.9. Assume (A I) and (A2). Then, for each hEX., the original
equation (14) is uniquely approximation-solvable.
Corollary 34.10. Assume (AI). Let A: X -+ X. be u'1rorml)' monotone and
continuous, and leI C: X -+ X. he compact. Then, for each real ;" :F O. the
operator
A(A + C): X -+ X.
;s A-proper.
PROOF.
(I) Admissible inner approximation scheme. From II En II = I it follows that
"E: II = 1 for all n. Since (X,.) is a Galerkin scheme, dist(u, X n )  0 as
n  oc for all u E X. This implies IIRnu - ull -+ 0 as n -+ x. That is the
compatibility condition (4).
(II) Consistency. Since A is continuous, the consistency follows from
Corollary 34.3.
(III) Stability. By (A2), for all u, t' EX,
< A u - A v, u - L') > a ( "u - [''')'' u - (,' II.
For all u, l' E X n' this implies
IIAn u - Anrllil u - I'll > (An u - An[',u - v)
= (All - AI'. Enu - Enl') = (Au - Ar, u - v)
> a(lIu - vll)lIu - vII.
Hence
IIAn u - Anvil > a(lIu - I'll)
for all u, t' E X n'

974 34. Inner Approximation Schemes, A-Proper Operato and the GaJerkin Method
(IV) Solvability. It follows from Theorem 26.A that, for each b e X the
equation Au = b, U E X, has a solution.
(V) By Theorem 34.A, the operator A: X -+ X. is A-proper and the equation
Au = b, U E X, is uniquely approximation-solvable.
Corollary 34.10 follows from Corollary 34.6.
o
34.6. Application to the Numerical Range of
Nonlinear Operators
We want to apply the main theorem on strongly stable operators (Theorem
34.8) to the operator equation
Au - ).14 = b,
u eX,
(17)
for fixed A E IK. Our objective is to find a set JI such that, for each j. e ...If and
each be X, equation (17) has a unique solution. In this connection, the
numerical range of A plays a fundamental role.
Definition 34.11. Let A: X -+ X be an operator on the H-space X over 0( = R,
C. The set
{ (U - vlAu - Av) }
%(A) = II l :u,veX,u#=v
u - vII
is called the Ilunierical range of A. Obviously, .K(A) is a subset of 0<.
EXAt.IPLE 34.12. Let A: X .-. X be linear and continuous. Then:
(a) .......(A) contains the eigenvalues of A.
(b) The closure of .,A;(A) contains the spectrum of A in the case where K = C.
(c) If). E ".(A), then Ii.I s; II A II.
(d) The set .Ar(A) is convex (Theorem of Hausdorff).
PROOF. Ad(a).lf Au = lu and 14 :t- 0, then (uIAu)/lIuIl 2 = ;" and hence;' e Ar(A).
Ad(b). Cf. Corollary 34. 14(a) below.
Ad (c). cr. Example 34.13 below.
Ad(d). A proof of this classical result can be found in Stone (1932a. M).
o
EXAMPLE 34.13. Let A: X ... X be Lipschitz continuous, i.e.,
IIAu - Avll  LUu - vII for all 14, VEX.
Then A e ,"'(A) implies lIt s L.

Problems
975
PROOF. This follows from
I (u - v I Au - At') I  II u - (' 1111 Au - A v II < L II u - (' 11 2 · 0
Theorem 34.C (Zarantonello (1964)). Suppose that:
(i) The operator A: X  X is continuous on the separable H-space X over
IK = R, C. We set A). = A - AI.
(ii) The number A E K has a positit)e distance from the numerical range of A.
We set d = dist(A, ,.''"(A)), i.e., d > O.
Then, for each b E X, the original equation (17) has a unique solut;on.
Corollary 34.14. Assume (i) and (ii). Then:
(a) The inverse operator Ail: X  X is Lipschitz continuous, i.e.,
II Ail b - Ail bll  d-Illb - bll for all b, bE X. (18)
(b) If A(O) = 0, then .¥(A) contains the eigenvalues of A.
(c) If, in addition, dim X = 00, then, for each b EX, the original equation (17)
is uniquely approximation-solvable.
PROOF. We use Theorem 34.8. For all u, v E X with u  v, we obtain the key
inequality
(u - t'IAu - Av)
l(u-vIA1u-A1t')I= A- Ilu-vIl 2 > dllu-vI1 2 , (19)
lIu - vll 2
i.e., A J.: X  X is strongly stable. This implies
IIA;.u - A;.vll > dllu - vII
for all u, v EX.
(20)
(I) Existence and uniqueness.
Let dim X = 00. Then AJ.u = b is uniquely approximation-solvable by
Theorem 34.8.
Let dim X < 00. As in Step 1 of Section 34.3, it follows from (20) and
the invariance of domain theorem that AJ.: X -+ X is bijective.
(II) Lipschitz continuity of Ail. Relation (18) follows from (20).
(III) If Au = AU, u #= 0 and A(O) = 0, then (uIAu)/lIuIl 2 = A, i.e., A E A'.(A).
o
PROBLEMS
34.1. Proof of Corollary 34.3.
Solution: By the compatibility condition (4),
IIE..R..u - ull-+O
as n -+ 00.
By assumption, the operator A is continuous. Hence
IIAE..R..u - Au!! -+ 0
as n -+ OC 1 .

976 34. Inner Approximalion Schemes, A-Proper Operators. and the Galerkin Method
Now, from sup"IIQ,,1I < 00, we obtain the consistency condition
IIQIIAu - Q"AE"R"ull  IIQlIlIlIAu - AE"Rllull -.. 0
34.2. Proof of Corollary 34.4.
· Solution: Use (I) and (III) in Step I of Section 34.3.
34.3. Proof of Corollary 34.5.
Solution: Use (III) in Step 1 of Section 34.3.
34.4. Proof of Corollary 34.6.
Solution: For brevity, we write n instead of n'. Let sup"lIu,,1I < 00 and let
as n -. 00.
lim IIQ,,(A + C)E"u lI - Q"bll = o.
,,-;x.
Since sup"IIE,,1I < 00, the sequence (Ellu lI ) is bounded. By assumption, the
operator C' is compact. Thus, there is a subsequence, again denoted by (14 11 ), such
that
C £"14,, -t Z in Y
as n -.. 00.
(21 )
From sup"IIQIIII < Cc it follows that IIQIICElluli - Q"zU -.. 0 as n -. 00. This
im plies
lim IIQIIAE"u" - Q,,(b - z)1I = o.
"-00
By assumption, the operator A is A-proper. Thus. there is a subsequence, again
denoted by (14,,), such that
E"u"  U in X
as n -. fX)
(22)
and Au = b - z. By (21) and (22), Cu = z. Therefore, (A + C)u = b, i.e., A + C
is A -proper.
34.5. Cont'ergence of projection operators. Let X I C X 2 C . eo be a sequence of linear
closed subspaces X" of the H-space X such that X = U" X". Let PIS: X -. X" be
the orthogonal projection operator from X onto X".
34.5a. Show that, as n -t 00, 14"  U implies u" - P"u  O.
Solution: Let 14"  u. For all ", E X,
(u" - P"uf w) = (u,,1 w) - (P"ul w) -+ 0,
since P"u -. u. This implies U" - P"u  O.
34.5b. Show that, as n -+ 00, 14" -+ u implies P"u" -+ u.
Solution: Let u" -t u. Then
IIP"u" - ull s IIP"u" - P"ull + IIP"u - ult
 II PIt 1111 U" - u II + 1/ P" 14 - u II -+ O.
Note that IIP"II = I if X  {O}.
34.6. An extremal problem. Let X" be a finite-dimensional linear subspace of the
B-space }(. Show that, for fixed U E X, the minimum problem
II u - 14" II = min!,
u ll EX",
(23)
has a solution.

Rclcrenocs 10 the Literature
977
Solution: Since 0 E X.. it is sufficient to consider problem (22) for all "II E
XII \\.ith . u,,11  lIu{. This modified problem has a solution b)' the classical
Weierstrass theorem, since dim X" < 00.
References to the Literature
Classical survey article on the applications of functional analysis to numerical mathe-
matics: Kantorovic (1948
Classical papers on A-proper maps: Petryshyn (1967), (1968), (1970). Bro\\'der and
Petryshyn (1969,.
Extensivc survey article on A-proper maps and their applications: Petryshyn
(1975. B).
Survey on the historical development: Petr)'shyn (1975), Schumann and Zeidler
( 1979).
A-proper maps. fixed-point theory, and eigenvalue problems: Petryshyn (1975. S
Fitzpatrick and Pctryshyn (197S (1978), (1979), (1982 Petryshyn (1987).
A-proper maps and the numerical stability of the Galerkin method: Petryshyn
( 1975).
A-proper maps and nonlinear elliptic differential equations: Petryshyn (1975). (1976
(1980), (19803). (1981).
A-proper maps and periodic solutions of ordinary differential equations: Petryshyn
(1986, S). (19800). Petryshyn and Y u (1985) (periodic motions of satellites).
Approximation schemes and numerical functional analysis: Cea (1964), Bro\\'der
(1967), (1968/76. M). Michlin (1969, M) (numerical stability of the Ritz method),
Sturnmel (1970/72), Temam (1970. M). (1977, M Anselone (1971, M), Aubin (1972,
M), Grigoriefl'(1973), Glowinski, Lions. and Tremolicrcs (1976, M), Ciarlet (1977, M),
Vainikko (1976. L), (1978. S). (1979. S Glowinski (1984, M), Deimling (1985, M),
Reinhardt (1985. M).
Nonstationary problems: Raviart (1967), Temam (1968), (1977, M), Girault and
Ra viart (1986. M).
Collectively compact operators and integral equations: Anselone (1971, M), Fenyo
and Stolle (1982, M), Vol. 4.
Projection methods: Krasnosclskii (1973. M).
Eigenvalue problems: Krasnoselskii (1973. M). Osborn (1975), Vainikko (1976, L),
( 1977), (1979, S).
Variational inequalities: Glowinski. Lions. and Trcmolicrcs (197 M).
Applications to mathematical physics: Glowinski. Lions. and Tremolieres (1976, M),
Ciarlet (1977, M). Ternam (1977, M). Glowinski (1984, M). Girault and Raviart
(1986, M).
The functional analysis of discretization methods: Vainikko (1976.  H), (1978, S),
(1979, S).
Numerical range: Bonsall and Duncan (1971. M) (recommended as an introduction),
Stone (1932a, M), Zarantonello (1964 Rhodius (1977).

CHAPTER 3S
External Approximation Schemes,
A - Proper Operators, and
the Difference Method
The most practical solution is a good theory.
Albert Einstein
The devil rides high on detail.
In order to elucidate the basic ideas, \VC consider the boundary value problem
for a quasi-linear elliptic differential equation of order 2m:
L (-I)JJIDClAG(x,Du(x» = f(x) on G,
121 S III
DPu(x) = 0 on cO
for all fJ: 1111 S; m - 1.
(I)
The corresponding difference nJethod reads as follows:
L (-l)bIVA.(P, V+u(P» = f,. (P) for all
pI  '"
P e ". III'
u,,{P) = 0
for all P II ".",.
(2)
In contrast to (I), the partial derivatives D- are replaced by difference quotients
V t -
Here, G denotes a bounded region in R".. In connection with the difference
method, we consider a lattice having grid width h. The precise definition of
m." will be given in Section 35.4. Roughly speaking. !I",.II consists of a]1 interior
lattice points of G that are at a distance greater than or equal to mh from the
boundary lattice points corresponding to the boundary oG of G. Thus it
immediately follows from
U,.(P) = 0 for all P j ..It
that
V! r4,,(P) = 0 for all boundary lattice points and for all fJ: IfJl < m - 1.
This condition corresponds to the boundary condition in (I).
978

External Approximation Schemes. A-Proper Operators. and the Difference Method 979
One obtains f" by using an integral mean value.
The appearance of the distinguishable difference quotients V + and V_is
explained in a naturai way if one goes over to the generalized statement of the
problem.
(A) The gelJeralized differential equation problenJ. Let X = W,'"(G). i.e., X is
a Sobolev space. We seek a function u e X such that
f. L A.(.,,Du{x»D«v(x)dx = f. f(x)v(x)d.
G I  at G
(8) The gelJeralized difference equation problem. Let XII = 1/F;'(fd,. i.e., X,.
is a so-called discrete Sobolev space. We seek a lattice function I'" e Kit such
that
f L A«{P, Vu,,(P»VaV,.(P) dx" = f J,.(p)V"(P) dx"
pI s; III
for all VEX. (1*)
for all v" eX".
(2.)
We abbreviate V + to V. Here, J ... dx,. means discrete integration, i.c., one sums
over all lattice points P and dx" = h N , where N is the dimension of G.
One notes here that (2*) follows from (t*) if one systematically makes the
follo\ving very natural substitutions:
(i) The region G goes over into the set of lattice points.
(ii) The functions u on G arc replaced by the lattice functions u" that are
defined in the lattice points.
(iii) Differential quotients D« are replaced by difference quotients V 2 .
(iv) The integrals JG. . . dx go over into the discrete integrals J . . . dx,..
(\') f is replaced by appropriate mean values J".
(vi) Sobolev spaces go over into discrete Sobolcv spaces.
As we shall see in Section 35.5, problem (2) follows from (2*) by discrete
integration by parts. For that reason, we need both V_and V + in (2).
In Section 26.5 we have proved that, under suitable assumptions, there
exists exactly one solution of (1*). The problem arises of showing the con-
vergellce of the corresponding difference method.
We solve the problem of convergence in this chapter by proving, parallel
to the preceding chapter, an abstract main theorem for external approxima-
tion schemes (Theorem 3S.A) and then applying this theorem to the difference
method (2*). In this connection, it is important that the differential equation
problem (1*) leads to an operator equation
Au = b,
ue X,
where the operator A: X -. X. is uniformly monotone.
Another possibility for an approximate solution of the differential equation
().) consists in using a Galerkin method with finite elements as basis functions.
For a suitable choice of the finite elements according to A 2 (60), the Galerkin
equations have the form (2*) in special cases. However, in the general case,
the simple difference method (2*) does not arise here.

980 35. External Approximation Schemes. A-Proper Operators, and the DiJrerenoe Method
The main ingredients of our approach in this chapter arc the synchroni-
zation condition (7) below and the discrete convergence u" .!. u as well as the
discrete- convergence u: !: u. of functionals.
35.1. External Approximation Schemes
Together with the initial problem
Au = b,
\ve consider the discretizcd problem
UE X,
(3)
A"u" = bIt'
u. EX",
n= I,...,
(4)
with the corresponding approximation scheme:
F f (3 X A. X*
lR'
X A. .
" ) X,..
(5)
The operator R,,: X  Xa is called a restriction operator and En: XII -. F is
called an extension operator. We call CJJ a synchronization operator. The
elements of F that lie in w(X) are said to be synchronized. The so-called
compatibility condition plays an essential role:
EaR"u ..... CJJ(u) in F as n -. oc and for all II EX, (6)
and also the sYllchron;zariolJ conditio'l:
The weak limits in F of the sequence {EnulI} and their
subsequences are synchronized, i.e., if
E..u". g in F as n..... 00,
(7)
then g e w(X).
EXAttfPLE 35.1. We show how to realize the external approximation scheme (5)
with respect to our concrete problem (1*), (2*). The statements will be made
precise in Section 35.6.
Let (h,,) be a sequence of positive numbers with hIt -. 0 as n -. 00. Here, hIt
dcnotes the grid width of the latticc. We choose:
(a) X is the Sobolev space W,'"(G).
(b) X,. is the discrete Sobolev space 1Y;'(9JIf..)'
(c) We assign to each U E X the tuple
w(u) = (D:lu" s...
that consists of u and all partial derivatives of u up to order m. Here,
DOu e Lp(G)
for all a: 1«1  m.

35.1. External Approximation Schemes
981
Therefore, C1J(U) lies in the corresponding product space, which we denote
by F, Le., we have w(u) E F.. where
F = n L,(G).
pf s '"
Consequently, the space F consists of all the tuples
(u"IS'" with"., E L,(G) for all .
The synchronized clements oo(u) of F have the special property that
r4 = Dtl u for all IX: 1«1 s m and fixed u e X.
(d) By means of an averaging process, the restriction operator R" assigns to
each U E X a lattice function "II E XII on the set "" of grid points.
(e) The extension operator E" describes an extension process for lattice func-
tions. In order to explain this, let u. e X" be a lattice function. We first
construct the tuple of difference quotients
(V".)Ism
and extend all VUII to Lp(G)-functions 11« on G. Then we define
E.u,. = (us))l1 S III.
We use the so-called external space F in order to be able to choose this
extension process s;mpl). It is essential that we do not require that E.u" =
(1I:1ls", is synchronized.
Let u" be a solution of the difference equation (2*). The role of the syn-
chronization condition (7) consists in that the weak limit of (E"u..) is syn-
chronized and yields a solution of the corresponding differential equation (1*).
In this connection, we will use the following important fact:
The extended difference quotiellts converge weakly in L,(G) 10 tile corre-
."pondi"g generalized derivatives.
This principle is the ke}' to our approach.
The approximation scheme described in Example 35.1 turns out, in Section
35.6, to be an admissible external approximation scheme in the sense of the
following definition.
Definition 35.2. The approximation scheme (5) is called an admissible external
approximation scheme iff the following hold:
(i) X, F, XII are real B-spaces with dim X" < 00 for all n e N, where F is
renexivc.
(ii) The operator (I): X ..... F is linear, continuous, and injective.
(iii) All the operators R,,: X --. X" and En: X" -+ F are linear and continuous
with supnUR,,1I < 00 and sup"IIE,,1I < cc.
(iv) The compatibility condition (6) and the synchronization condition (7)
above hold.

982 35. External Approximation Schemes, A-Proper Operators. and the Difference Method
An inner approximation scheme of the form (34.16) arises as a special case
when f" = X and C1J is the identity.
In Theorem 35.A in the following section, we will require that the operators
All and A of the external scheme (5) are rclatcd to each othcr by a weak
consistency condition. However, in contrast to the inner approximation
scheme in Chapter 34, we do lJot require that the equation A" = E: AE" holds.
To describe the convergence of the difference method we use the following
discrete convergence:
II Un - R"ullx -.0
n
To be precise, we have the following definition.
d
Un -+ U
iff
as n -+ 00.
(8a)
Definition 35.3. Let (un> be a sequence of elemcnts with Un e X n for all n e N.
The sequence (un) converges discretely to u e X iff
lim II Un - Rnullx = o.
"
" -. OCI
W . II
e write Un -+ u.
The following convergence concept for functionals is decisive for thc proof
of convergence for the difference method:
u:u. iff (u:,un)Xn-+(u.,u)x as n-+oo. (8b)
To be more precise, the following definition of discrete. convergence holds.
Definition 35.4. Let (u:) be a sequence offunctionals with u: e X: for all n e N.
The sequence (u:) converges discretely. to u. e X. iff
lim (u:, u,,)x n = (u., u)x
n-oo
holds for all sequences (un)' U" E X"' with sUPnllu"lIxn < 00 and
E"u"  w(u) in F as n -+ 00.
We write u:  u*.
35.2. Main Theorem on Stable Discretization
Methods with External Approximation Schemes
We investigate the connection between the following conditions.
(CI) Solvability. For each bE X, the original equation
Au = b
,
U EX,
has a solution.

35.2. Main Theorem on Stable Discretization Methods
983
(C2) Unique approximation-solvability. The following hold for all b E X*:
(i) The equation Au = b has a unique solution U E X.
(ii) For each bit E X: and all n  "Ot the approximate equation
A"u" = bIt
has a unique solution u" e XII'
(iii) As II -+ 00,
bIt  b
implies
..
Ult -t> U
and E"u"  oo(u) in F.
(C3) A-properness. The operator A: X -+ X. is A-proper with respect to the
external approximation scheme (S)t i.e., by definition the following holds:
.
A.... u,,' -+ b
and sup".lIulI,lIx < 00 imply the existence of a subsequence (U"II) with
"
d
u.. -+ u and Au = b.
In this connection (II') denotes an arbitrary subsequence of the sequence
of natural numbers and we suppose that U,,' eXit' for all n'.
The following theorem is the main result of this chapter.
Theorem 35.A (Schumann (1979». The "Jree conditions (Cl)t (C2 and (C3) are
mliluall)' equit'alent in the case where the following hold:
(H]) Approximation scheme. We are given the admissible external approxi-
mation schenJe (5). All the operators A" in (5) are conti,uIOUS.
(H2) Weak consistency. For all u e X
.,-
AnR"u -+ Au.
(H3) Stability. For all u, v E X.. and all n > no,
ItA"u - A..vllx.  a(flu - vllx ),
" "
",here the g;f,'en function a: [0, ex> [ -+ R is strictly monotone increasing and
continllolls \vith a(O) = 0 and
a(t) -+ +00
as t -+ +cc.
(H4) Approximation of the right-hand term b. For each b e X*, there exists
a sequence (bit) \\lith
.,.
b.  b,
\vhere b. e X: for all n > no.
The significance of this theorem for the applications in Section 35.5 consists
in that, for the difference method, conditions (H 1) through (H4) can easily be
verified for quasi-linear elliptic differential equations. In this connection,
(H2) results from the convergence theorems for the Lebesque integral. It is

984 35. External Approximation Sc A-Proper Operators- and the Difference Melhod
important that no a priori estimates whatsoever are needed for the difference
quotients.
35.3. Proof of the Main Theorem
The follo\ving preparatory lemma is proved in Problem 35.1. The synchroni-
zation condition is used here in (ii) and (iii).
Lemma 35.5. Tile following hold for an admissible external approximation
scheme as n .... oc:
(i) u..!. u implies E"ll" -. W(ll) in F.
(ii) u:  II. implies sup" 11 u: II x: < OC).
(Hi) u:  0 inJplies II u: II x.  o.
"
(iv) EnR"ll -. llJ(U) .(or all U e U implies E..R"u -+ w(u) for all U e X provided U
is dense in X.
We no\\' give a proof of Theorem 35.A that is parallel to that of Theorem
34.A.
Step 1: (C3) implies (C2).
(I) For fixed b n E X:, equation A.u" = bIt has exactly one solution U" e X..
for all n  no. This follows analogously to Section 34.3.
(II) We show that, for fixed b e X., equation Au = b has at most one solution
u eX.
Let AI4 = At'. It follows from (H3) that
a(IIR"u - R"vll) < IIA"Rnu - A"R"vll for all n. (9)
By (H2),
d.
A"Ra u - A"R"v -t O.
Lemma 35.5(iii) yields IIA"Rnu - A"R"vJl .... o. Therefore. by (9),
IlR"u - R"v!J  0 as n -. X).
From this it follows that
IIEaR"u - E"R"vn S (SUp" II E"II) II R"u - Rnvll .... 0
as n -+ ct;,'.
The compatibility condition (6) yields w(u - v) = 0; therefore, u = v.
(III) We show that, for each b e X., the equation All = b has exactly one
solution u e X. To this end, we choose a sequence b,,!: b according to
(H4). Furthermore, we choose u" with A"u n = bIt according to (I).
By (H2), it follows from A"R"(O)  A(O) and Lemma 35.S(ii) that
sup.IIAnR,,(O)1I < 00. Because R,,(O) = 0, the following holds for all n:
IIball = IIAnu,,1I  IIA"u" - A"(O)II - IIA"R..(O)II
> a( (Ju"lI) - II A"R,,(O)II.

35.4. Discrete Sobolcy Spaces
985
Therefore. sup" a(Uu"lI) < 00 and hence sup.llu"U < 00. The A-properness
of the operator A ensures the existence of a subsequence (u",) with
4
14,,' --. 14 and Au = b.
(IV) We show that b.  band A"u.. = b" imply u"  u and E"u. .-. w(u) in F.
By (III), we obtain: Each subsequence (14".) of (14,,) has another sub-
sequence (u"..) with Il,,"  u and Au = b. The limit element u is the same
for all subsequences since Au = b has exactly one solution u. This implies
the convergence of the entire sequence, i.e., we get 14"  u. Indeed, this
follows by using an analogous argument as in the proof of the con-
vergence principle (Proposition 10.13(1». By Lemma 35.S(i), II"  II
implies £..14" -+ (1)(14).
Step 2: (C2) implies (C 1): This is trivial.
Step 3: (CI) implies (C3).
For brevity, we write n instead of n'. Let
4-
A..u" -+ b
\vith sup"llu,,1I < 00. We choose a point u with Au = b according to (Cl). We
want to show that
II
U.. -+ u.
Then the operator A is A-proper with respect to the approximation scheme
(5), i.e., condition (C3) holds. Indeed, by (H2),
4-
A"R"u -+ Au.
This implies A.14" - A"R"u  O. Lemma 35.S(iii) yields
II Anu.. - A..R"ull .-. 0 as" -. 00.
By (H3), a(lIu" - R,,14U) < IIA"u" - A"R"ull --.0 as n -+ 00. This implies
lIu" - R.ull .-. O. i.e., U"  14.
The proof of Theorem 3S.A is complete. 0
35.4. Discrete Sobolev Spaces
We now make ready the applications of Theorem 35.A to partial differential
equations.
To this end, we choose a cube lattice in R'-' with the grid mesh h. The
coordinates of the lattice points P are integer multiples of h. To each lattice
point P we assign a cube c,,(P) with edges parallel to the axes having edge
length hand P as midpoint. In this connection, we choose C,.(P) to be,
say, half-open and in fact so that the union of all cubes yields a disjoint
decomposition of R'''. Let G be a bounded region in IR''I with sufficiently
smooth boundary. i.e., oG E Co. I .

986 35. External Approximation Schemes. A-Proper Operators, and the DifFerence Method
aG
. .
Figure 35.1
Defi nition 35.6. We understand It to be the set of all lattice points P with
c,,(P} c G. These lattice points are called interior lattice points of G. The cubes
belonging to " approximate G from the interior.
By the set of boundary lattice points o" we understand all lattice points of
, that belong to the boundary cubes. These are in a natural way the cubes
whose closure docs not lie entirely in the interior of the cube set belonging to
".
For m = 1,  . . . , by ,... we understand the set of all lattice points of" that
have distance from the boundary lattice points greater than or equal to mh.
In Figure 35.1, the points and the small circles mean the lattice points
belonging to ,. while the points mark the boundary lattice points of ofd,..
Moreover, the small circles mark the lattice points of ".1.
By a lattice function u.. we understand a function that assigns a real number
to each lattice point of AN.
Definition 35.7. We define the difference quotient VtU,,{P) to be
V t (P) _ u,,(P :t he;) - u,,{P) (10)
i U, - - :J: h ·
In this connection. e, is the unit vector in the direction of the ith coordinate.
If P has the coordinates (hg l' · · . . hg.'i) where the 9 l' . . ., gN are integers, then
there results the point P :t hei if one replaces 9, by 9; :t I.
The difference operator Vl:. corresponds to the differential operator D i =
alai. Analogous to the differential operator
DtJ = &:1 · · · U:.f,
we define the difference operator
Vi: = (Vt )ClI · · · (Vi )tJ.
for tX = (alt.. .,a.v). For brevity, we agree to write
V. = V.+,
V tJ - V .
- +.
We now define discrete Lebesgue spaces and Sobolev spaces parallel to
Lp(G) and W,'"CG1 respectively. In this connection, we make use of the discrete

35.4. Discrete Sobolc\' Spaces
987
integral
f lI"dx"   u,,(P)hl\'.
Here, the summation is over all lattice points P of R N . The sum always exists
since the lattice functions to be considered are different from zero only in
finitely many lattice points.
Defmition 35.8. Let I  p < x), m = I, 2, ... . By the discrete Lebesgue space
,(,) we understand the set of all lattice functions that vanish identically
outside ,.. We choose the norm to be
lu,,\,. = (f lu,,\" dX,,) 1/,..
By the discrete Sobolev space 1Ir".(1t) we understand the set of all lattice
functions u" that vanish identically outside "',,,. We choose the norm to be
lu,.I.., = (f L lu,.IP dX,, ) II" ·
1«: Sift
Moreover, we define
I u,.I.".o = (f L l\12u,I" dX, ) II" ·
I czt .. 1ft
Proposition 35.9 With the given 1I0r"JS, p(") and 1Y;'(,,) are real B-spaces.
The nornas 1'1...., a lad 1'1... ,.0 are eqllivalent on 1I1tt(,,).
We deal with the proof in Problem 35.2. Note that the norms are defined
in a way completely parallel to the norms on L,(G) and W,"'(G). Parallel to
integration by parts there is also a formula for discrete integration b)1 parts:
f IIV1vdx" = (-1)1«' f (V  u)vdx..
(II)
Proposition 35.10. Formula (II) holds for all u, v E ;/r".(..), where 1(%1 :s; m.
We recommend that the reader do the proof as a simple exercise. In
conclusion, we mention an extension method that will play an important role
in the next section.
Definition 35.11. Let u" be a lattice function. By a lattice function extended to
the region G, which we shall also designate by U"t we mean:
( ) _ { U,.{P} for x E C,.(P). P e ,..
u.. x - 0 otherwise.

988 35. External Approximation Schemes. A-Proper Operators. and the Difference Method
This way u" is extended in a natural way as a constant to each cube that
belongs to an interior lattice point, i.e., u" is piecewise constant. Obviously,
fe; u" dx = f u" dx".
In the following, the integral appearing on the left-hand side of this equation
is always to be understood in this sense.
35.5. Application to Difference Methods
In order not to obscure the simple basic idea by purely technical details, we
consider only a simple example. We treat the general situation of quasi-linear
elliptic differential equations of order 2m in Problem 35.4.
We investigate the boundary value problem
N
- L D;(ID;uIP- 2D i U ) + su = f on G,
i=1
( 12)
u = 0 on (1G,
with the corresponding difference equations
N
- L V;-(IV;u,,(P)lP- 2 V;u,,(P)) + su,,(P) = l,,(p)
it: I
for all P E ",
u,,(P) = 0
for all P E a".
( 13)
We make the following assumptions.
(A) G is a bounded region in R N , N > I, with sufficiently smooth boundary,
i.e., oG E co. I , and s is a nonnegative real number. We choose a sufficiently
small positive number ho so that the set " of interior lattice points is not
empty for all h, 0 < h  hoe We understand J,,{P) to be the integral mean
value of f over the cube c,,(P) belonging to P, i.e.,
J,.(P) = h- N f f(x)dx. (14)
Ch(P.
Definition 35.12. Let X = Wpl(G), X" = ;i''(,,), 2  p < 00, p-l + q-I = t.
The generalized problem for (t 2) reads as follows: For a given function
f E Lq( G), we seek a function U E X such that
a(u, v) = b{t,) for all v EX, (t 2)
with
a(u,v) = f ( .f IDjuIP-2DjuDjV + SUV ) dX,
G .= I
b(v) = fG!VdX.

3S.S. Application to Difference Methods
989
The generalized problem for (13) reads as follows: We seek a lattice function
"II E X,. such that
a,.(u,., V,.) = b,.(v,.)
for all I'" e X,._
( 13*)
with
a,,(u,,_ v,,) = f C IV j u"IP- 2 V i u" Vjv" + SU"v,,) dx",
b,,(v,,) = f v" dx".
Proposition 35.13. Under the assunaplion (A) abot'e, the follo,,';ng three asser-
tiolts are t'alid:
(a) Differential equation. The generalized probleln for (12) has exact I}' one
solr4t ion u.
(b) Difference equation. The generalized problem for (13) lJas e:(Qctly one
solution u ll J'Or all h. 0 < h  hoe
(c) Convergence. As II --. +0, ti,e seqllelJce (14,) conl'trges to u in the !ollo,,'i'JlJ
sense:
J. ( t ID4" - V 4 u"IP + I" - u"l p ) dx -+ 0, (15)
G 1=1
L ( t \V j " - V,""I P + I " - ""II' ) IJ/\' -+ o. (16)
p f: ". I i-I
In this connection, (15) and (16) describe the convergence of the difference
method on the region G and on the lattice, respectively. In (IS), 14,. and Viu,.
denote the lattice functions extended to G in the sense of Definition 35.11. In
(16), for brevitYt we write u, and u instead of U,.(P) and u(P), where u (P) is the
mean value of u at the point P in the sense of (14). Moreover, Vi u and Vi""
stand for the difference quotients V, u (P) and ViU,.(P) of the lattice functions
P...... u(P) and P....... u,,(P), respectively.
Corollary 35.14 (Equivalence). The difference equation (13) aluJ the correspond-
ing generalized problem (13*) are mutually equit'illenf.
PROOF OF COROLLARY 35.14. Multiplication of(13) by v,., discrete integration,
and discrete integration by parts yield (13-). Furthermore, (13) follows from
( 13 *) by a reversal of this proced ure. 0
Therefore, instead of (13*), we need to solve only the nonlinear system of
equations (13). This happens with the aid of the following iteration method
for k = 0, I, . . . .:
U"+l)(P) = u1"t(P) - Igp(U")
ut+l)(P) = 0 for P e iJ",
for P e 'B". 1 ,
(17)

990 35. External Approximation Schemes, A-Proper Opcrato and the Difference Method
with the starting elements u1°)(P) = 0, where
N
gp(u,,) = - L VI-(IV,u..(P)I'-zV,u,.(P» + su,,(P) - f,, (P).
i"l
Corollar)' 35.15. Let 0 < h S ho and s > O. Then, for sufficientl}' smal" > 0,
tile iteratiol' metlJod (17) converges as k -+ 00 to the solution u,. of tile difference
equation (13).
8y Theorem 26.8, one can specify explicitly the domain of t values. The
proof will be given in Problem 35.7.
35.6. Proof of Convergence
We want to prove Proposition 35.13. To this end, we apply Theorem 35.A
and simply verify the hypotheses (HI)-(H4) and (CI). Then the statement of
Proposition 35.13 is precisely (Cl) in Theorem 35.A.
Step I: Condition (HI).
We construct the following approximation scheme:
A X .
t
F  rR.
A.
X It ) X..
(18)
To this end, let (h.) be a sequence of positive numbers with h. -+ 0 as n -+ 00
and 0 < h.  ho for all n E . We set
X = Wpl (G), X. = .';,,1 (fd",.),
N
F = n Lp(G).
f-i
2  p < 00.
Moreover. we set U lt = u,.,.. We equip X. \\rith the norm U-U = 1.1 1 ,p.o.
The s}'nchronization operator w: X -. F is defined by
w(u) = (u, D. u,..., DHu).
The restriction operator R II : X -+ X" results from forming the mean value, that
is, we set k = hit and we define
k-N f. u(x)dx for PE..I'
(Rllu)(P) = CkCP)
o for P , ", 1 ·
Furthermore, we define the extension operator Ell: X" .... F by
Ellu. = (14", VI 14",. . - , V HU,,),

35.6. Proof of Convergence
991
where the lattice functions extended to the region G occur on the right (cf.
Definition 35.11). This way we obtain Ellu. e F.
Lemma 35.16. The diagranl (18) represents an admissible external approxima-
tion scheme.
The proof which uses standard arguments on discrete Sobolev spaces can
be found in Schumann and Zeidler (1979). In this connection, by Lemma
35.5(iv). one needs to verify the compatibility condition
EnR"u -. w(u) in F as n... 00
only for all U E Ct(G) since CO(G) is dense in X.
The synchronization condition is obtained as follows. Let
E"u,,-:.g in F
as n -+ cc
with g = (u, U I ...., U".). From this it follows that
UnI' in Lp(G) as n-+oo,
Vtu n .... Vi in L,(G) as n.... 00.
It is a known fact that the extended difference quotients converge weakly to
the generalized derivatives (cf. Temam (1970, M), p. 69). Hence,
U. = D.u
. .
and therefore, g = w(u).
Step 2: The operator A and the condition (CI).
By Proposition 26.10, there exists an operator A: X .... X. with
(Au, v) = a(ll, v) for all u. veX.
The generalized problem (12.) is equivalent to
Au = b,
ue x.
(19)
Note that Ie L 4 (G) with q-l + p-J = I implies be X*. Furthermore, by
Proposition 26.10, equation (19) has exactly one solution lIe X. This is (Cl).
In addition, it follows from Proposition 26.10 that
a(u,u - v) - a(v,u - v)  cllu - vllf.,.o + s fG (u - v)2dx, (20)
for all u, veX and fixed c > o.
Step 3: The operator A. and the condition (H3).
Analogous to the proof (II) of Proposition 26.10, there results
a(u, u - v) - a."(v, u - v)
 clu - vlt"o + s f (u - V)2 dxll"
for all u, veX.. (21)

992 35. External Approximation Schemes, A-Proper Operators. and the Dilferen(e Melhod
In this connection. replace the derivative D. by the difference quotient Vi and
the integrals by discrete integrals. For u e X,., the mapping
v.-. ala (u, v)
"
is a linear functional on the space XII and, because dim X,. < OCJ. it is also
continuous. Therefore, there exists an operator A,.: XII -. X: with
(Allu, v) = a" (u. v) for all u, v e Xn.
"
This implies
IIA,.r4 - A,.vllllu - vn > I<A,.14 - A"v,14 - v)1 > cllu - vII'
and hence
IIA ll f4 - Anvil > cllu - v1l P - 1 for all u, ve X,._
This is precisely the stability condition (H3).
The generalized discrete problem (13*) is equivalent to the operator equation
A,.14,. = bIt'
u,. EX,."
(22)
where we write bIt for bIt .
"
Step 4: The weak consistency condition (H2).
We must show that
.,.
A"R"u -+ AI4
By definition" this is equivalent to
a"n(R"u, v,,) -+ a(u, v) as n  00, (23)
for all sequences (VII) with the property v,. e XII for all n E N as well as
sup" II v,,11 < 00 and
for all u EX.
E,.v,. -JII. w(v) in F
as n -+ 00.
(24)
Explicitly, relation (23) means that, as n -+ 00,
fG (IViii"IP-1Vi U" Vit.'. + S U. V.)dX -+ fG (ID;UIP-2D,UD,V + SUV)dX,
(25)
where U,. denotes the lattice function arising from the function u eX, for all
grid points P e "".1' by forming the mean values according to (14). More
precisely, in relation (25), the symbols u" , V, u,. " V". V.v" denote the correspond-
ing extended lattice functions in the sense of Definition 35.11. Recall that
u" = u. .
n
Let us prove (25). From
E,.v,.w(v)
as n -+ CX)
it follows that. as n ... 00,
v v
,.
and
v, v..  D,v in L,(G).
(25a)

35.6. Proof of Convergence
993
The compatibility condition E"R"u -+ w(u) means that, as n -+ OC],
U -+ u
"
and
Viii" -+ DiU in L p ( G).
(25b)
Since q < p, the embedding Lp(G) C Lq(G) is continuous. This implies that, as
n  'Y.J,
alII -+ u and Viii" -+ DiU in Lq(G). (25c)
By Proposition 26.6, the Nemyckii operator v......., vl P - 2[, is continuous from
Lp(G) to Lq(G), since p/q = p - I. Thus, it follows from (25b) that, as n -+ 'XJ,
IV i u"l p - 2 u" -+ ID;ul p - 2 Diu in Lq(G). (25d)
From (25a, C, d) we obtain (25), by A 2 (35).
Step 5: Condition (H4).
d.
We have to show that bIt -+ b. Recall that
h(t,) = fG ft' dx for all (' E X.
Since we write b n for bit , we have
"
b,,( I'll) = fG J,." ('II dx
for all t'n EX",
(26)
where fit denotes the mean value in the sense of (14).
We need the following lemma.
Lemma 35.17.// Ie Lq(G), then hit -+ I in Lq(G) as n -+ 00.
We leave the proof to the reader as an exercise.
Now let (v,,) be a sequence with V n E X" for all n E N such that supnllrnll < 
and
E"l'"  w(v) in F
as n -+ oc;.
This implies
I'"  f' in L p ( G)
as n -+ 00,
and hence
h n ( f; n) -+ b ( l' ) as n -+ oc:,
by Lemma 35.17 and A 2 (35). This means that bPI  h.
Step 6: Proof of Proposition 35.13.
By Theorem 35.A, the condition (C2) formulated there is identical to
Proposition 35.13. In particular, the statements (15) and (16) follow from
E n ll"  w(u) in F
as n  00
d d .
an lI" -+ u, I.e.,
lIu n - Rnullx -+ 0 as n -+ 00.
"
In fact, this implies (15) and I u" - Ii Il. p . 0 -+ 0 as n  00, and hence we get (16).

994 35. External Approximation Schemes, A-Proper Operators, and the DifTerenl."e Method
In this connection, note that ii -+ u in Lp(G) as n -+ 00 by Lemma 35.17, and
hence U" -+ Ii in Lp(G) as n -+ 00 by (15), i.e., I U" - u I p -+ 0 as n -+ 00.
The proof of Proposition 35.13 is complete. 0
PROBLEMS
35.1. Proof of /jemma 35.5.
Solution: Ad(i). By (6) and (8a), it follows from u"  U that, as n .... 00,
II E"u" - w(u) II < II E"u" - Ell R"u II + II E" R"u - (o(u)1I
 (sup,,11 E"II> lIu" - R"ull + II E"R"u - w(u)1I -t O.
Ad(ii). Let u:  u.. If sup"lIu: II < 00 does not hold, then there IS a
subsequence, again denoted by (u:), with
Ilu:II > n
for all n.
Beca use
lIu:1I = sup{ (u:,u,,): lIu,,1I = I},
there is a subsequence, again denoted by (u,,), such that
(27)
II u"" = 1
and
(u:,u,,) > n
for all n.
(28)
Because sup" II E"II < OC:, we have sup,,11 E"u" II < 00. Since the B-space F is
reflexive, perhaps after going over to a subsequence, we get
E"u"  9 in F
as n.... 00.
The synchronization condition (7) yields g = w(u). Thus, u:  u. leads to
( u: , u,,) -+ (u., u)
as n -t 00.
This contradicts (28).
Ad(iii). Let u:  o. By (27), there exists a sequence (un) with lIu,,1I = I
and
III u: II - < u:, u,,) I < I In
for all n.
As in (ii), there exists a subsequence, again denoted by (u,,), such that
E"u"  w(u) in F as n.... 00.
Since u:  0, we obtain
(U:, u,,) .... 0 as n -t 00,
and hence II u: II -. 0 as n -. 00.
In fact, we have shown that every subsequence of (lIu:11) possesses a sub-
sequence which goes to zero. From this it follows that the entire sequence (lIu: II)
goes to zero according to the convergence principle (Proposition 10.13).
Ad(iv). Let E"R"u .... w(u) as n -. 00 for all u E V, where U is dense in the
B-space X. We want to show that, for all v E X,
E"R"v -+ w(v)
as n.... 00.
To this end, we use an approximation argument. Let v E X and t: > 0 be fixed.

Problems
995
Then we obtain
IIE"R"v - w(v)D  IIE.R.v - E"R"u: + IIE.R.u - w(u)1I + IIw(u) - w(v)1I
S (sup. Ii E"II IIR.II + IIwq)lIv - ull + II E"R"u - w(u)1 < t,
for all n  no(e in the case where we choose u e U so that II u - v II is sufficiently
small.
35.2. Proof of Proposition 35.9.
Solution: Let XII = 11.(,.). Since X. is a tinite-dimensional space, and all
norms are equivalent on such spaces, it suffices to verify that 1'1.." and 1'1",.".0
are norms on X..
We note that lu..I",." = 0 automatically implies II" == O. Moreover.lu"I..,.o =
o yields that the mth order difference quotients vanish identically. However,
since u" vanishes at the lattice points of a sufficient)' large boundar)' strip, all
lower difference quotients also equal zero at the boundary lattice points. From
this it follows that u,. ;; o.
35.3. Proof of Proposition 35.10.
Hint: cr. Ladyzenskaja (1985, M), p. 221.
35.4.. Difference methods for quasi-linear elliptic differential equations of order 2m.
Generalize Proposition 35.13 to this situation.
Hint: Cf. Schumann and Zeidler (1979). It is crucial that the weak consistenc)'
condition (H2) in Theorem 3S.A can be verified easily. To this end. use the
principle of majorized convergence for the Lebesgue integral and the con-
vergence argument in Problem 26.4. The stability of the operator A. results
from the uniform monotonicity of the operator A which corresponds to the
differential equation.
35.5. Proof of Lemma 35.16.
Hint a. Schumann and Zeidler (1979).
35.6. Proof of Lemma 35.17.
Hint: a. Schumann and Zeidler (1979
35.7. Proof of Corollar)' 35.1 S.
Solution: We use Proposition 26.8. To this end, we must verif)':
L (g,.(I1,,) - g,,(v..))(u.(P) - v,,{P))  s L (II,.(P) - V,{P))2 (29)
, r
and
u...-. 9,.(u,,) is locally Lipschitz continuous. (30)
Let f(x) = Ixl,-2 x. P  2. Then (30) follows from the local Lipschitz con-
tinuity of f. Moreover. (29) follows from the monotonicity of /. i.e.,
(f(x) - f(y»(- - )')  o.
To be precise, after discrete integration by parts, (29) is identical to (21) in the
case where c == O.

996 35. External Approximation Schemes. A- Proper Operators. and the Difference Method
References to the Literature
Discrete Sobolev spaces: Raviart (1967), Temam (1970, M), (1977, M), Ladyzenskaja
(1973, M).
Theorem 35.A and applications to quasi-linear elliptic differential equations:
Schumann and Zeidler (1979).
Difference methods for nonlinear elliptic differential equations: Brezis and Sibony
(1968), Aubin (1970), (1972, M), Sobolevskii and Tiuncik (1970), M liller (1974),
Schumann and Zeidler (1979), Hack busch and Trottenberg (1982, P), Hackbusch
(1985, M).
Multigrid methods and fast solvers: Hackbusch and Trottenberg (1982, P), Birkhoff
and Schoenstadt (1984, P), Hack busch (1985, M) (standard work).
Finite elements: Ciarlet (1977, M), Temam (1977, M), Giraull and Raviart (1986, M).
Quasi-linear elliptic equations and finite elements: Frehse (1977), (1982, S), Frehse
and Rannacher (1978), Dobrowolski and Rannacher (1980), Kufner and Sandig
( 1987, M).

CHAPTER 36
Mapping Degree for A-Proper Operators
The concept of degree of mapping, in all its different forms, is one of the most
effective tools for studying the properties of existence and multiplicity of solu-
tions of nonlinear equations.
Fclix E. Browder (1983)
In Part I we demonstrated the fundamental importance of the Leray-
Schauder mapping degree for operator equations io\'olving compact opera-
tors. In this chapter we will generalize the Leray-Schauder mapping degree
deg(l - C, G. b) for compact operators C: G c X -+ X to a mapping degree
DEG(A, G, b)
for A-proper operators A: G e X...  In this connection, we use the same
approximation process as in Chapter 12. However, in contrast to Chapter Il
now the mapping degree of the equations being approximated need nor tend
to a unique limit value; hence, DEG(A, G, b) is, in the general case, a set of
integer.4;.
Let X be a separable H-space over 0(. Then, by Section 34.4, all the
operators A: X  X of the form
A = l(B - K - C) (1)
are A-proper in the case where the following hold:
(i) B: X ..... X is continuous and strongly monotone (e.g., B = l that is,
Re(Bfl - Bvl fl - v)  c lIu - vll 2 for all u, veX and fixed c > o.
(ii) K: X -. X is Lipschitz continuous. that is. more precisely,
IIKfI - Kvll S dllu - vfl for all fl, veX
and fixed d with 0  d < c.
997

998
36. Mapping Degree Cor A-Proper Operators
(iii) C: X -. X is compact.
(iv) ). is a nonzero fixed number in IK.
Therefore, in particular, the general mapping degree DEG(A, G, b) includes
the following classes of operators:
(a) For ).. = 1, B = /, K = 0, we obtain in (1) the operators for which the
Leray-Schauder mapping degree is defined.
(b) For A. = 1 and B = 1. (1) contains the class A = I - K - C, where K is
k-contractive and C is compact, i.e., K + C is a k-set contraction.
The point of departure for our discussion is the approximation scheme in
Section 34.1, i.e., we use:
X
R. H 
A . y
1 Q..
A" = Q"AE".
(2)
x"
A,
. 
The basic idea of our approach is to approximate the operator equation
-
Au = b, U E G,
by means of the equation
Allu" = Q"b, u" e G II ,
where G" = E;I(G).
In order to simplify the exposition, we consider only operators A that are
defined on the entire space X. However, the considerations can also be easily
carried over to the case where A has the form A: G e X.... t: Then, in the
definition of A-properness, parallel to Section 34.2, we have to consider only
sequences from E;I( G ).
In Problem 36.3 we study in detail a mapping degree for demicontinuous
(S).. -operators. This mapping degree is based on the convergence of the
Galerkin method for (S)+-operators, which we have proved in Proposition
27.4.
36.1. Definition of the Mapping Degree
We make the following assumptions, where the condition
Au #: b for all u e aG
(3)
is crucial.
(H 1) The operator A: X -+ Y is A-proper with respect to the admissible inner
approximation schema (2) in the sense of Definition 34.2, and condition
(3) holds.

36. J. Definition of the Mapping Degree
999
(H2) G is a nonempty, open, and bounded subset of X. Let 0" = E;l(G). The
sets G" are uniformly bOIl,uled, i.e., there is an R > 0 such that 1111,,11  R
for all u.. e G" and all n. _
(H3) All the operators All are continuous on G n .
(H4) All the spaces X"' Y. are provided with a fixed orientation, i.e.. we
designate a fixed basis.
Proposition 36.1 Under the assulnptions (H I) llu-ough (H4), there exists a
number no Stlcll that the Brou\ver Inapping degree deg(A", G.., Q"b) exists for all
n  no.
We give the proof in Problem 36.1.
Proposition 36.1 enables us to make the following fundamental definition.
Definition 36.2. Let all = deg(A.. G", Qnb) for n  no. Under the assumptions
(H 1) through (H4), we set
DEG(A. G, b) = the set of cluster points of the sequence (all).
If G = 0, then we set DEG(A, G, b) = o.
Recall that the number a with -00 < a S oc is called a cluster point of the
sequence (an) iff some subsequence of (a,,) converges to a. Moreover. recall the
fact that the integer deg(A", G", Q.b) is a measure of the number of solutions
u" of the equation
A"u" = Q"b,
-
II" e G",
where, roughly speakin& the solutions are counted according to their multi-
plicity (cf. Chapter 12).
The definition ofDEG(A, G, b) goes back to Browder and Petryshyn (1969).
As the proof in Problem 36.1 shows, deg(A., G". Qllb) depends only on the
orientation of Xa and f", but not on the basis chosen.
EXA1tfPLE 36.3. Let X be a real H-space with dim X = :x>. Let G be a bounded
region in X with 0 e G. Then
DEG(-I,G,O) = {-I,I}
holds for -1, the negative of the identity. in the case where we choose the
approximation scheme (34.10).
PROOF. By (34.10), G,. = E;l(G) = G tl XII' It follows from formula (3) in
Chapter 13 that
deg( -I, G", 0) = (-I)", where n = dim X".
Now Definition 36.2 yields the assertion. 0

1000
36. Mapping Degree for A-Proper Operators
36.2. Properties of the Mapping Degree
All the properties of the Brouwer mapping degree in Part I can easily be
generalized to DEG(A, G, h). We give several examples of this.
Proposition 36.4 (Existence Principle). If DEG(A, G, b) ¥- {O}, then tile equa-
t;on Au = b has a solut;on u E G.
PROC)f. Let an = deg(A II , G", Qllb). If a E DEG(A, G, b) with a  0, then, by
definition, there exists a subsequence, again denoted by (all)' such that all -+ a
as n -+ 00. Consequently, a" ¥- 0 for all n > no. By the existence principle (D2)
of the Brouwer mapping degree in Section 13.6, we obtain from all  0 that
the equation
Allu ll = Qll b ,
U" E G II ,
has a solution 14 11 for all n > '1 0 . By assumption (H2) above, the sets G" arc
uniformly bounded, i.e., sUP1i1lu1I1I < 00. The A-properness of the operator A
yields the existence of a subsequence, again denoted by (u lI ), such that Ellu ll ..... U
as '1 -+ OCI and Au = b. 0
Proposition 36.5 (Homotopy Principle). We have
DEG(A o , G, b) = DEG(A l' G, b)
in the case ,,,here the following hold.
(i) There exists a family {A,} of operators A,: X -+ Y for all t E [0, I] such
that A, satisfies the assumptions (H I) through (H4) in Section 36.1 for all
I E [0, 1].
(ii) The mapping (u, t)  A,(u) ;s cont ;nuous on G x [0, I].
(iii) The mapping t  A,(u) is conti,luoUS on [0, I] uniformly with respect to
U E G, i.e., for each E; > 0 and .for each t E [0, 1], there exists a beE, t) > 0
su that II A,(u) - AJ(u)1I < t holds for It - sl < b(E, t) and U E G.
We give the proof in Problem 36.2.
36.3. The Antipoda! Theorem for
A-Proper Operators
Theorem 36.A (Antipodal Theorem). Let b E  The equation
Au = b,
U E G,
(4)
has a solution u in the case "'here tIle following hold along with aSSllmpt;ons

36.4. A General Existence Principle
1001
(H I) through (H4) in Section 36.1:
(i) Au #= tb for all u e aG, t e [0, 1].
(ii) A( -II) = - A(u) for all u E aG.
PROOF. We set B, = Au - tb. The operator A is A-proper. By Corollary 34.6.
this is also true for the compact perturbation B, of A. Proposition 36.5 yields
DEG(B o , G, 0) = DEG(B 1 , G,O).
We now consider the operator A" = Q"AE". Note that oG Ii c E;I(oG) and
that the operators Eft and Q,. are linear. Thus, it follows from (ii) that Aft is
odd on oGft. The classical antipodal theorem (Theorem 16.8) yields
deg(Aft' G.., 0) :F O.
By Definition 36.2, DEG(A, G,O)  {O}. Since Bo = A, we obtain
DEG(B 1 , G, 0) #= {O}.
Now, Proposition 36.4 yields the existence of a solution of the equation
8, u = O. u e G. This equation is equivalent to the original equation (4). 0
36.4. A General Existence Principle
As an another example we generalize the important fixed-point principle in
Section 13.1. In this connection, the following condition on the omitted rays
is crucial:
Au :Fe -au
for all u e oG and all real   O.
(5)
Theorem 36.8. The equation
Au = 0,
ue G,
(6)
has a solution in the case ",here ti,e follo,,,;ng assumptions are fulfilled:
(i) X is a separable injinite-dimelJSional H-space, and G is an open bounded
set in X ,,,ith 0 e G.
(ii) Tile operator A: X -+ X is bounded, continuous, and condition (5) holds.
(iii) For all«  OJ the operator A + a.l ;s A-proper with respect to the approxi-
mation scheme (10) in Chapter 34.
Corollary 36.6. Condition (5) is satisfied in the case where
Re(Aulu) > 0 for all u e aGe
J\loreover, condition (iii) is satisfied in lhe case ",IIere ti,e operator A possesses
the structure (1).

1002
36. Mapping Degree ror A.Proper Operators
PROOF. According to the approximation scheme (34.10), we obtain QII = P"
and Q"l E" = 1 on XII. By property (Dl) of the mapping degree in Section 13.6,
deg(Q"IE.,G",O) = deg(I,G",O) = 1.
This implies
DEG(/, G,O) = {I}.
We now consider the homotopy
A,II = (1 - I)AII + tu.
For t #: 1, we obtain
A, = (I - t)(A + 1(1 - 1)-11).
Thus, by (iii), the operator A, is A-proper for all t e [0, 1]. According to the
key condition (5), we have
A,"  0 for all (u, t) e aG x [0, 1].
The homotopy principle (Proposition 36.5) yields
DEG(Ao, G,O) = DEG(A I' G,O).
Since Ao = A and AI = I, we get
DEG(A, G,O) = {I}.
Now, according to the existence principle (Proposition 36.4), equation (6) has
a solution. 0
PROBLEMS
36.1. Proof of Proposition 36.1.
Solution: By (H4) we can identify X. and Y. with (R4I. The mapping E,,: XII .... X
is continuous. Therefore, the set E;I(G) is open and E;I(G) is closed. Con-
sequently, the set G" is open. bounded, and nonempty for all n  n 1 and
iJG" S E;I(oG).
We show that there exist ad> 0 and an no such that
IIQ"AE"u. - Q.bll  d
(7)
for all U" e oG. and all n  110. Otherwise, there exists a sequence (u..) with
U.' e cG.. for all n' and
OA..u.. - Q,,bll ... 0
as n -+ 00.
Moreover, by (H2), sup..llu..11 < 00. The operator A is A-proper. Consequently.
there exists a subsequence denoted by (u.) such that
E" u" ..... u in X
as n  00
and Au = b. Because E"u. e 00 for all n, we have u e oG. This is a contradiction
to Au :I: b for all u e oG.

Problems
1003
From (7) we obtain
A"u" =F Q"b
for all u" E G", n > no.
(8)
The operator A,,: X" -t Y" is continuous. Now, according to Chapter 12. the
existence of the Brouwer mapping degree deg(A", G", Q"b) follows from (8).
The proof of Proposition 36.1 is complete.
By Step I in the proof of Theorem 12.B, the mapping degree deg(A", G", Q"b)
does not change if one goes over to other bases in X" and Y" which have the
same orientation as the bases fixed by (H4).
36.2. Proof of Proposition 36.5.
Solution: In (7) we replace the operator A by A, and we show that the numbers
d and. no can be chosen uniformly for all I E [0, I]. By Problem 36.1 one first
finds,. for each I E [0, 1]. numbers de,) and "o(t). Because of the continuity of
t t-t A,(u) on [0, I], uniformly with respect to U E at and sup,," Q"II < 00. one
finds, for each t E [0, I], a neighborhood U (t) such that d and "0 can be chosen
uniformly on U(t). The set [0, I] is compact. Therefore, finitely many U(l;)
already cover the interval [0.1]. Now choose d = min;{d(t,)} and no =
max, {nO(t i )}.
We set
H,,(u, t) = Q"A,E"u,
From (7) with A, instead of A we obtain
H(u, t) = A,(u).
H,,(u, t) # Q"b
for all (u, t) E oG" x [0, 1] and n > no. The homotopy invariance (D4) of the
Brouwer mapping degree in Section 13.6 yields
deg(H,,( ',0), G". Q"b) = deg(H,,(', I). G", Q"b)
for all n > "0' By Definition 36.2, it follows from this that
DEG(A o . G, b) = DEG(A I' G, b).
36.3. Mapping degref for demicontinuous bounded operators with condition (5)+. Let
X be a real separable reflexive B-space with dim X = 00, and let G be a
nonempty bounded open set in X. Moreover. let .f/ denote the set of all bounded
demicontinuous operators
A: X.... X.
which satisfy (S)+. For example, the operator
Au = Mu + Cu + b
belongs to!/ in the case where the operator M: X -+ X. is uniformly monotone,
demicontinuous, and bounded, the operator C: X -+ X. is compact, and b
is a fixed element in X.. Let {"-'I'W 2 ....} be a basis in X and let X" =
span {Wi..... w,,}. We choose functionals w,. E X. such that (K ' i ., J) = b.) for all
I, }.
We want to solve the equation
Au = 0.
U E G,
(9)

1004
36. Mapping Degree for A-Proper Operators
by means of the Galerkin equation
A"u. :11II 0,
U" E G..
(10)
where G" = G n Xa and
.
A.u = L (Au'''J)xK1.
r1
36.3a. Lemma. Show that if A e !/ and Au :#: 0 on aG, then there exists an no such that
A"u  0 on oG" for all n;:: 110.
Solution: Otherwise, there exists a sequence (u",), bricOy denoted by (14.). such
that
A"u. = O.
u. e oG.
for all n  no.
Since (u,,) is bounded, there exists a subsequence, again denoted by (II,,). such
that
u" ...... 14
as n..... 00.
We choose a sequence (v.) with v" E X" for all n such that ". --+ u as n .... 00. Then
(Au". u" - 14) :: (Au., f'a - u)
for all n  no.
since (A.u", w) - (Au". w) for all we X" and A.u" = o. Hence
(Au". u" - u) -t 0
as n -. 00,
since (Au.) is bounded and v" .... 14 as n .... 00. Condition (5)+ implies 14"  U as
n  . Hence 14 e aG and Au = O. This is a contradiction.
36.3b. Defillition. Let A E !/ and suppose that Au #: 0 on iJG. We define
deg(A. G) = deg(A". G,,) for all n  no.
Show that this definition does not depend on the choice of n and the basis
{"'I. w 2'...}.
Solution: Use exactly the same argument as in the proof of Theorem 12.8
concerning the Leray-Schauder degree.
36.3c. Existence principle. Show that if
deg(A, G) :;: 0,
then the equation Au :: 0, U E G, has a solution.
Solution: By definition of the mapping degree, deg(A", G,,)  0 for all n  no.
According to the existence principle for the Brouwer mapping degree, the
Galerkin equation (10) has a solution u. E G" for all n > no. By Proposition
27.4. there exists a subsequence (14 11 ,) with u,,- .... U as n  a::: and Au I: O. Hence
14 e G. Since Au #: 0 on aG, we get U E G.
36.3<1. Additivity. Let
.
G = U t
.-1
where {J\f_} is a family of pairwise disjoint open bounded sets. Suppose that

Problems
1005
A E ..l/ with
Au  0 on oG
Show that
and
Au :I: 0 on aM.
for all i.
.
deg(A, G) = L deg(A, M,).
i=1
Solution: This follows from the corresponding propert)' of the Brouwer
mapping degree.
36.3e. HOmOIOp}' ;nt1lU;ance. Let H: X x [0. 1] -+ X. be a dcmicontinuous bounded
operator with condition (S). x [0, I], i.e., from
U. -II. U
and
I.. ... t
as n... oc:,
with tIt e [0, 1] for all n, and
lim (H(u". I,,). u. - u) < 0
"-.00
it follows that u"  u as n .-. 00. Suppose that
H (u, t)  0 on cG x [0, 1].
Show that
dcg(H(-.O).G) = deg(H(., 1). G).
Solution: Use a similar argument as in Problem 36.2.
Note that H(', 0) and H(', I) belong to Y.
36.3f. Linear honJotopy. Let A, B E tl'. Set
H(u, t) = (1 - t)Au + tBu
for all (u, t) e X x [0, I]
and show that H satisfies condition (S). x [0, 1]_
Solution: Let u"  u and I. -+ I as n .... 00 with
lim (I - t.>(Au.,u" - u) + t"(Bu..,u,, - u)  O. (11)
,,-
Since A is demicontinuous and satisfies (S)..., we have
lim (Au", u. - u)  O.
8-00
Otherwise, there would exist a subsequence, again denoted by (u,,), such that
lim (Au., u. - u) < O.
,,-
Condition (S).. implies u" ... u and hence Au....... Au; therefore, (Au", u - u.> .... 0
as n  . This is a contradiction.
Suppose. without loss of generality, that t > O. From
lim (I - t")(Au.,u,, - u)  0
" -. 00
and (II) "'C obtain
I lim (Bu". u" - u) = lim 1"(Bu,,. u" - u) S O.
" - oc.
. .. ox
Condition (5).. implies U" .... u as n ... 00.

1006
36. Mapping Degree for A-Proper Operators
36.3g. Convex;')' of the class f/. Show that if the operators At B: X -. X. belong to
the class f/ in the sense of the definition given above. then A + B E !/ and
AA e !I' for each;' > O. In panicular, A. Be !/ implies (I - l)A + IS e .9' for
each t e [0,1], i.e., /1' is convex. Moreover, if we set C(u) c - A( - u) for all
u e X, then A e 9' implies C e 9'.
Solution: Use the same argument as in Problem 36.3f and use the definition
of (S). in Section 27.1.
36.3h. An existence theorem. Let A e  and suppose that
(Au,u) > 0 for all u e aGo (12)
Show that if 0 e G, then deg(A, G) = 1. and the equation Au = 0, u e G, has a
solution.
Solution: Condition (12) implies that Allu  - u on iJG" for all oc  O. By
Theorem 13.A, dcg(A., G,,) = 1 for all n  110- Now use Problems 36.3b, c.
36.3i. Antipodal theorem. Let G = {u E X: lIuli < r} and let A e !/. Suppose that Au 
o on cG and
Au A( -u)
IIAull  IIA( - u)
Then deg(A, G) is odd and the equation Au = 0, u e G, has a solution.
Solution: We use the linear homotopy
for al1 u e oG.
(13)
H(U,I) = (I - r)Au + I(A(u) - A(-u».
(14)
lienee
deg(A, G) = dcg(II(., I G).
Note tbat H(u, t) #= 0 on oG x [0, I]. by(t3). ThUs. (14) represents an admissible
homotopy by Problems 36.f, g.
Since II( - u, J) = - H(u, I), the classical antipodal theorem (Theorem 16.8)
yields
deg(H,,( · , 1), G,,) ;: odd for all n  no.
This completes the proof by Problems 36.3b, c.
Furtber details on this mapping degree can be found in Browder (1968/76,
M) (e.g. applications to pscudomonotonc operators) and in Skrypnik (1986, M).
In particular, it is shown in Browder (1983) that, parallel to Chapter 12. this
mapping degree is uniquely determined by a few natural axioms.
Applications of this mapping degree to quasi-linear elliptic differential equa-
tions are studied in detail in Skrypnik (1986, M).
36.4. The nonlinear open mapping theorem. We assume:
(i) Let G be an open set in the real separable reOexive B-space X.
(ii) The operator A: G -.. X" is continuous and locally injective.
(iii) The operator A satisfies the condition (5). on G (e.g., A E 9). That is. it
follows (rom u" -.... u as n -+ 00 on G and
lim (Au. - Au,u. - u)  0
II-aC
that U II -+ U as n -+ 00.
Show that the set A(G) is open in X..

References to the Literature
1007
Hint: Use the same argument as in the proof of Theorem 16.C from Part I
based on the antipodallheorem. Replace the Leray-Schauder degree by the
mapping degree introduced above. cr. Skrypnik (1986, M). p. 45.
36.5. The inuariance of domain theorem. Assume (i) through (iii) from Problem 36.4.
Show that if G is a regio then so is A(G).
Solution: The set G is open and connected. By Problem 36.4, the set A(G)
is open. Since A is continuous. it sends connected sets onto connected sets
(AI (13c»). Hence A(G) is connected, i.e., A(G) is a region.
The fundamental importance of the open mapping theorem and the invari-
ancc of the domain theorem has been thoroughly discussed in Section 16.4 from
Part I.
References to the Literature
Mapping degree for A-proper maps: Browder and Petryshyn (1969), Bro\\'der(1968;76,
M), Petryshyn (1975, S, B, H), (1980).
Mapping degree Cor demicontinuous (S). -operators: Browder (1968/76, M)t (1983),
Skrypnik (1973, M), (1986, M).
Applications to quasi-linear elliptic differential equations: Skrypnik (t 973. M), (1986,
M).

Appendix
In contrast to the classical Riemann integral, my new concept of the integral
provides a better answer to the question of the connection betv,'een differentia-
tion and integration (main theorem of calculus).
Henri Leon Lebesgue (1902)
Almost all concepts, which relate to the modern measure and integration
theory, go back to the works of Lebesgue (187S-1941 The introduction of these
concepts was the turning point in the transition ftom mathematics of the
nineteenth century to mathematics of the twentieth centul)'.
Naum Jakovlevi Vilenkin (1975)
The purpose of this Appendix is to collect a number of important well-known
analytical tools. This is done for the convenience of the reader. We consider
the following topics:
(a) The Lebesgue measure on R''1.
(b) Measurable functions.
(c) The Lebesgue integral for functions with values in B-spaces.
(d) Lebesgue spaces.
(e) Sobolev spaces.
(f) Galerkin schemes in 8-spaces and the method of finite elements.
(g) Ordinary differential equations with measurable functions.
(h) Distributions and locally convex spaces.
(i) Construction of general measures and the main theorem of measure
theory.
(j) General measure spaces.
(k) The integral with respect to general measures.
(I) Measures on topological spaces.
(m) The classical Stieltjes integral and Sticltjcs operator integrals.
1009

1010
Appendix
(0) Measures and the spectral theory for self-adjoint operators in H-spaces.
(0) The functional calculus for self-adjoint operators in H-spaces.
(p) linear semigroups and fractional powers of operators.
(q) I nterpolatioo theory.
(r) Hausdorff measure, Hausdorff dimension, and fractals.
(s) Functions of bounded variation.
These tools will be used frequently in Parts 11- V. Further basic material can
be found in the Appendix of Part I. In case the opposite is not stated explicitly,
all B-spaces are assumed to be real or complex B-spaces.
Lebesgue Measure
(I) Measurable sets. The Lebesgue measure is a generalization of the vol-
ume in elementary geometry. The classical volume was only defined for a small
class of sufficiently regular sets. The goal of Lebesgue's measure theory around
1900 was to find a sufficiently large class of sets S in AN which can be measured
by a number meas S, where
o < measS S 00.
Such sets are called Lebesgue measurable or briefly measurable. The precise
definition of the Lebesgue measure will be given in (75h) below as a special
case of a general construction (main theorem of general measure theory). The
most important properties of measurable sets in R N are the following:
(a) The measure of cuboids is equal to the classical volume.
(b) Open sets and closed sets are measurable.
(c) The union or the intersection of at most countably many measurable sets
is again measurable.
(d) If {S.} is an at most countable family of pairwise disjoint measurable sets,
then
meas ( y s) =  meas Sj.
(e) The difference S - T of two measurable sets Sand T is again measurable.
(f) A subset S of R N has measure zero iff, for each £ > 0, there exists a family
of at most countably many cuboids C. such that S c U, C. and
L meas C i < t.
I
Examples. A set of finitely many points or of countably many points in R N
has measure zero.
Sufficiently regular curves in (R2 or R J have measure zero.
Sufficiently regular surfaces in R J have measure zero.

Measurable Functions
1011
The set of all rational numbers in R 1 is countable and hence it has measure
ze ro.
The set of all points in R.\' with rational coordinates has measure zero.
(2) "Almost every",'here." By definition, a property P holds true almost
everywhere iff P holds true for all points of n N with the exception of a set of
measure zero.
One also uses "almost all." For example, almost all real numbers are
irrational.
The importance of the notion "almost everywhere" is shown by the follow-
ing theorem.
(3) Theorem of Lebesgue. Let f: J £; R  R be a monotone function on the
finite or infinite interval J. Then, f is almost everywhere differentiable and
therefore, almost everywhere continuous.
According to the famous Hungarian mathematician Fryges (Frederick)
Riesz (1880-1956), this is one of the most surprising theorems of modern
analysis.
Note that most sets in R N are measurable. The construction of non-
measurable sets is based on sophisticated arguments.
Measurable Functions
Let Y be a 8-space.
(4) Step functions. A function f: M c R N  Y is called a step function iff f
is piecewise constant. To be precise, we suppose that the set M is measurable
and that there exist finitely many pairwise disjoint measurable subsets M; of
M such that meas M j < 00 for all i and
{ a.
f(x) = o.
for x E M j and all it
otherwise.
(5) The integral of a step function is defined to be
f f d.'( = L (meas Mj)a i .
M i
The general definition of the Lebesgue integral in (14) below will be based on
this simple definition.
(6) Example. Let f: [atb] -+ R be a step function as in Figure I. Then the
integral in the sense of (5) is precisly the classical integral.
(7) Definition of measurable functions. The function
f: M c (RN  Y

1012
Appendix
.
f
/ .
. (
J-- - --. .\
a h
Figure I
with values in the B-space Y is called measurable iff the following hold:
(i) The domain of definition M is measurable.
(ii) There exists a sequence (f,,) of step functions f,.: M -+ Y such that
lim .t;.(x) = f(x) for almost all x E M.
" - iX)
(8) Standard example. The function f: M c R N -+ Y is measurable if the
following hold:
(i) M is measurable and the B-space Y is separable.
(ii) f is continuous almost everywhere, i.e. t there exists a set Z of measure zero
in (RN such that j": M - Z -+ Y is continuous.
(9a) Calculus. Linear combinations, norm functions, and limits of measur-
able functions are again measurable.
More precisely, we set
F(x) = a(x)f(x) + b(x)g(x),
H(x) = lim j,,(x),
G(x) = IIf(x) II ,
" .... I
and we suppose that the functions
f, g, fIt: M £ (RN -+ Y,
a, b: M -+ R
are measurable for all n. Moreover, we assume that the limit f,.(x) -+ f(x) as
n -+ 00 exists for almost all x EM.
Then the functions F.. G.. and H are measurable.
(9b) Modification of measurable functions. If we change a measurable
function at the points of a set of measure zero, then the function remains
measurable.
In particular.. the function H in (9a) may be defined arbitrarily at the points
at which the limit does not exist.
(10) Theore,,, of Pettis. Let Y be a real separable B-space and let f: f c
(RN -+ Y be a measurable function. Then the following two statements are
equivalent:
(i) The function f is measurable"

The Lebesgue Integral for Functions wilh Values in B.Spaccs
1013
(ii) The real functions .,...... (g,f(x» are measurable on M for all functionals
g e Y*.
This important result reduces the measurability of functions with values in
B-spaces to the measurability of real functions. We will frequently use this
theorem.
(11 a) The theorem of Luzin alld the characterizatioll of measurable functions.
Let .r: M s; IR". -+ R be a function on the measurable set M with meas M < 00.
Then the following two statements are equivalent.
(i) f is measurable.
(ii) I is continuous up to small sets, i.e., for each  > 0, there exists a subset
lyl of M such that
f is continuous on the closed set M - M and me as M, < .
(II b) The cOllvergence tlJeorem of Egorov. Each convergent sequence of
measurable functions on a set of finite measure is uniformly convergent up to
small sets.
To be precise, let C(,.) be a sequence of measurable functions f,,: J\I 5 R It . -+
Y with meas M < 00 such that
f,,(x) -+ f(x)
as n -+ oc,. for almost all x E M.
Then the function f: M -+ Y is measurable and, for each b > 0, there exists a
subset M of J\f such that
In ::t f as n -+ 00 on M - M6 and meas Md < .
(12) De/i'Jition of measurable functiolls via substitution. We set
F(.) = f(X,14(X».
If the function u: J'4 c R N -. U is measurable, then the function F: JW .... Y is
also measurable provided the following assumptions are satisfied:
(i) The set M is measurable and the B-spaccs U and Yare real and separable.
(ii) The function f: J\f x U -+ Y satisfies the Caratheodory condition, i.e.,
x t-+ I(x, u) is measurable on M for all II e U,
u t-+ f{x, u) is continuous on U for almost all x e M.
The Lebesgue Integral for Functions with Values in B-Spaces
Basic definitions in mathematics must be simple.
F olclore
Let Y be a B-space. We consider the function
f: M c RN ... Y

1014
Appendix
on the measurable set M. The definition of the integral is based on the very
natural formula
(13) f f dx = lim r f"dx
.V ..... 00 J AI
together with the following two simple formulas:
(13a) I(x) = lim f,,(x) for almost all x e J\f t
n-CJC
I, nf,.(x) - f",(x)1I dx < £
Here. the functions f,,: M -. Yare step functions.
( 13b)
for aU  m > no(t).
(14) Definition of the integral. The function f: M -t Y is called jlltegrable iff
J'I is measurable and there exists a sequence (f,.) of step functions f,.: M -+ Y
such that (13a) and (13b) hold.
To be precise. we demand that, for each € > O there is an noel:) such that
(13b) holds.
The integral for integrable functions is defined by (13) above.
(IS) Jrlstification of the definition. By (14 the integral is well defined
because:
(i) the integrals over step functions have been defined in (5); and
(ii) the limit in (13) exists in Yand does not depend on the choice of the step
functions f".
According to (13a), each integrable function is measurable.
Conditions (13a) and (13b) mean intuitively that the sequence (f,,) of step
functions approximates f with a sufficiently high accuracy.
The value of the integral f AI f dx does not change in the case where the
function f is changed on a set of measure zero.
(16) Standard example. The integral J." f dx exists if the following two
conditions are satisfied:
(i) The function f: JW c RJ\. ..... Y is measurable (e.g.. Y is separable and f is
continuous almost everywhere in the sense of (8».
(ii) SUPXE" II/(x)" < 00 and meas M < 00.
Note that
J" f dx E Y.
The classical Lebesgue integral corresponds to the special case Y = R i.e., the
existence of J M I dx implies
f.., f dx < 00
and
o  measJW  00.

Properties of the Integral
1015
Properties of the Integral
Let Y be a B-space.
(17) Majorant criterion. All the following integrals exist and we have the
estimates
fM ldX < f,,, II/(x)/ldx < f", gdx
provided the following two conditions are satisfied:
(i) II f(.:( ) /I < g (x) for a I m os t all x E AI, and f AI Y d x e x is ts.
(ii) f: M c R N .... Y is measurable.
(18) Norm criterion. Let f: M c (RN -. Y be measurable. Then
f M I(x) dx exists iff f M II/(x)1I dx exists.
(19) Majorized convergence. We have
lim r I" dx = f lim J(x) dx,
"ocJM 1.1"-00
where all the integrals and limits exist, provided the following conditions are
satisfied:
(i) Ilf,,(.\:)1I < g(x) for almost all x E M and all n E N, and J 1.1 g dx exists.
(ii) lim"... x f,,(.x) exists for almost all x EM, where .f,,: M c IR N .... Y is measur-
able for all n.
This theorem of Lebesgue describes one of the most important properties
of the integral and will be used frequently. The key condition is the majorant
condition (i).
(19a) Generalized nlajorized convergence. Theorem (19) remains true if we
replace the assumption (i) with the following more general assumption:
Ilf,,(x)1I < g,,(x)
for almost all x E M and all n E N.
All the functions g", g: M .... R arc integrable and we have the convergence
g" -+ 9 almost everywhere on M as n -+ oc along with
f", g"d."( -+ fM gdx as n -+ 00.
Proof. Let f(.) = lim,,_oc: f,,(x). Hence IIf(x)1I < g() and
g(x) + g,,(x) - IIf(x) - };'(x)1I > o.
In the following we write f instead of f(x), etc.
By the majorant criterion (17) and the lemma of Fatou (19c) below, we

1016
Appendix
obtain
f 2gdx < ! f(g + g" - III - 1"II)dx
= f 2gdX - lim f II! - J;.I! dx.
"-XI
This implies lim n -<Xi f II! - J;.II dx = 0, i.e.,
f (f - J;.)dx < f III - J;.I! dx -.0 as n -. 00. 0
(19b) Monotone convergence. We have
lim f .(" dx = f lim J;.(x) dx,
" - C() !tI !tI ,,- <:()
where all the integrals and limits exist, 1 provided the following two conditions
are satisfied:
(i) f,,: M £; R N -+ R is integrable for all n E N.
(ii) The sequence (J;.) is monotone increasing (or monotone decreasing) and
sup" IJ",J;.dxl < 00.
(19c) Lemma of Fatou. We have
f lim !,,(x) dx < lim f J;. dx,
'" "-00 "-<I) !tI
where all the integrals exist, 2 provided the following two conditions are
satisfied:
(i) J;.: M c R N -+ R is nonnegative and integrable for all n E N.
(ii) lim,.-oo f", J;. dx < 00.
This lemma generalizes the well-known relation
lim a" + I tm b"  l. im (a" + b,,)
"-00
,,-
,,-
for nonnegative real numbers a" and b".
(20) Absolute continuity of the integral. Let f: M c (RN -+ Y be integrable.
Then, for each £ > 0, there exists a c5(£) > 0 such that
fH I dx  fH II/(x)1! dx < £
holds for all subsets H of M with meas H < b(£).
I In particular, it follows that lim.... f..(x)  1: OCJ for almost all x E M.
1 In particular, it follows that 0 S lam. e:.r>.h.(X) < OCJ for almost all "( E M.

Iteratd Integration
1017
(21) Absolute eqllicolltinuit}' alulthe cont'ergence theorem of Vitali. We have
lim f f"dx = r lim .f..ex)dx.
II"' J M J JI ncx>
where all the integrals and the left-hand limit exist, provided the following
four conditions are satisfied:
(i) In: M £ tR It .  R is integrable for all n e N.
(ii) Iim"...,:£; f..(x) exists for almost all x e M and is finite.
(iii) Equicontinuity. For each t > 0, there exists a bet) > 0 such that
sup r 1f,,(x)1 dx < t
II JH
holds for all subsets H of JW with meas H < b(£).
(iv) If meas M = 00, then, for each t > 0, there exists a subset S of M such
that meas S < 0C1 and
sp l,-s If.(x)1 dx < e.
(22) The convergelJce theorena of Vitali-Hahn-Saks. Theorem (21) remains
true if we replace assumption (iii) with the following weaker condition:
(iii*) lim ll _ cc I" In dx exists and is finite for all measurable subsets H of M.
In addition, (Hi*) implies (iii).
Iterated Integration
(I)
Our goal is the fundamental formula
r jex.y) dx dy = f (f, !(X,Y)dY ) dX
J AI J RN R'.
= fRL (fRlrf(X.}')dX )d}'.
In this connection, \ve set f(x, y) = 0 outside M. Furthermore, let x e R'" and
y e R L .
(23) Theorem of Fubin;. Let!: M c R.'1+L -.IR be integrable. Then formula
(I) holds. To be precise, the inner integrals exist for almost all x € R''I (resp.
almost all )' e (RL), and the outer integrals exist.
(24) Theorem of Tonelli. Let f: M  RN+L -.(R be measurable. Then the
following two conditions are equivalent:

1018
Appendix
(i) The function I is integrable.
(ii) There exists at least one of the iterated integrals in (I) if f is replaced by
If I, i.e., J (J III dy)dx exists or J (J III dx)dy exists.
If condition (ii) is satisfied, then all the assertions of the Theorem of Fubini
(23) are valid.
Parameter Integrals
We consider the function
F(p) = 1,!(X,p}dX
for all parameters pEP. Here,
f: M x P -. R
is a function, where M is a measurable subset of R N and P is a metric space
(e.g., a subset of IRK).
(25a) Continuity. The function F: P -. R is well defined and continuous if
the following three conditions are satisfied:
(i) The function x 1--+ f(x, p) is measurable on M for all pEP.
(ii) There exists an integrable function g: M -. R such that
If(x, p)1  g(x)
holds for all pEP and almost all x E M.
(iii) The function p....... f(x, p) is continuous on P for almost all x EM.
(25b) Differentiability. Let P be an open subset of R. The function f": P -t R
is differentiable and
F'(p} = 1,!p(X,P)dX for all pEP
provided the following two conditions are satisfied:
(i) The integral J M I(x, p) dx exists for all pEP.
(ii) There exists an integrable function g: M -. R such that
1/,(x, p)1  g(x)
for all pEP and almost all x E M. (This condition tacitly includes the
existence of the derivative fp(, p) for all pEP and almost all x EM.)
Lebesgue's Main Theorem of Calculus
(25c) Main theorem. Let F: [a, b] -. R be a function, where -00 < a < b <
00. Then the following two conditions are equivalent:

Le besgue Spaces
1019
(i) There exists an integrable function f: [a, b] -+ R such that
F(x) = f..Jr.f(t)dt forall xe[a.b].
(ii) F is absolutely continuous.
Moreover, if (i) holds then
F(x) = f(x) for almost all x e [a, b].
(2Sd) Recall that F: [a, b] ... R is said to be absolutel)7 conti,u,ous iff, for
each £ > 0, there exists a b > 0 such that
 IF(b.:> - F(at)1 < £
holds for aU finite systems of pairwise disjoint subintervals [a., ba:J of [a, b J
with total length < b.
(2Se) Differential;olJ of integrals '\lith respect to the domain. Let f: J c
IAN -+ Y be an integrable function from the open set J\f to the B-space l: and
let C be a cube in R N with x e C and measure m( q. Then, for almost all x EM,
lim :q J. f(t)dt = f(.v:),
m(C)"'O m c
lim :q J. 11/(1) - /(X) II dl = O.
M(C)-'O m c
I n particular, these limit relations hold for all points x at which f is continuous.
Lebesgue Spaces
Let G be a nonempty bounded open set 1 in (RN, N  1.
(26) DefinitiolJ of the Lebesgue space L,(G). Let 1 < P < r£. We set
lIull" = (fG lul' dx ) I".
Let Lp(G) denote the set of all measurable functions
u:G-+R with IIr'lIp<oo.
Then Lp(G) together with the norm U. U, becomes a real B-space once we
identify any two functions which differ only on a set of J'J-dimensional
Lebesgue measure zero.
More precisely, the two measurable functions u, v: G -. R are called equi-
valent itTu(x) = v(.v:) for almost all x e G. Then, the elements of the space L,(G)
J Most of the follov,.ing statements remain true if G is an unbounded open set or. more generalJy,
if G is a noncmpty measurable set in R...., N  I.

1020
Appendix
are precisely all the equivalence classes of measurable functions u: G -. R with
respect to this equi\'alence relation.
The space L 2 (G) together with the scalar product
(ulv) = fG uvdx
becomes an A-space.
(27) L,(G) is separable and Ct(G) is dense in L,(G).
(28) If I < p < co. then the B-space L,(G) is uniformly convex and hence
reflexive.
(29) The Halder ineqlla/il y. Let 1 < PI' . . . ,Pit < 00 be given with Li-I pi 1 =
I and let "i e L,.(G) for all i. Then
J. It It (J. ) 111',
n U i d."#; < n I Ui II" d."( .
G ;=1 1=1 G
where all the integrals exist.
(30) Inaportanl inequalities for real numbers. Let I  S < 00, 0 < r < 00,
1 < p < OC', p-J + q-I = I. Then, for all nonnegative real numbers  I' ..., 1\"
, '1, we have the follo\\'ing inequalities:
( . ) IJI 1\' ( N ) I/
a L:  L i S; b L: ..
i.-I i-I i-I
( ,V ) ' N
i c;i S C i c;i,
.. p "t
'1 < - + -,
P q
N .  f'
n i  L --
f1 i-I PI
(30a)
(30b)
(30e)
(Young's inequality),
where Pi is given as in (29), and
.V ( N ) 1/ ( N ) 1/.
(30<1) j '''j S , f I ",
(Holder's inequality).
Here, the positive constants Q, b, c depend only on N, s, r.
These inequalities are frequently used in analysis in order to obtain esti-
mates for integrals.
(3Ia) Example I. Let I  q, Pi < 00 and
u e Lq(G), V, e L",(G)
for aU i.

Lebesgue Spaces
1021
From the estimate
I w(x)1  const (I u(x)1 +  I Vj(X) 1"' 4 )
for all x e G, it follo\vs that K' e L,(G) and
II w ll 4  coost ("UI4 + f IIVII:'4).
Proof. By (30b),
Iw(x)1 4  coost (1Il(X)1 4 +  IV1(X)I").
Integration over G yields
II wll: < const (II ulI: +  11 1 ',11::).
Now the assertion follows from (JOb).
(31 b) Exalllple 2. Let 1 < p < 00, p-I + q-t = I and
"I E L,(G), v. E L..(G) for all i.
o
Then
' J. .'1
L "iVi dx < L lIu i ll,l1 v ;n 4
1=1 G 1=1
( .'1 ) 1/1' ( .'1 ) 1/4
 coost  II UIII I II v,II: ·
This follows from the Holdcr inequalities (29) and (30e1).
(32) Mean conlinu;t)'. Let I  p < OC,;. Every function U E L,(G) is p-mean
continuous, i.e., for each £ > 0, there exists a b(£) > 0 such that
fG Iu(x + h) - u(x)IPdx < e
provided Ihl < cS(£). In this connection, we set u(x) = 0 outside G.
(33) The compactness cheoren. of Riesz-Kolmogorov. Let 1  p < oc and
suppose that S is a bounded set in L,(G). Then the following two conditions
are equivalent:
(i) S is relatively compact.
(ii) S is p-",ean equicontinrlous. i.e., for each £ > 0, there exists a (e) > 0 such
that
sup J. lu(x + h) - u(x)I'dx < E:
..s G

1022
Appendix
provided Ihl < (e). Here, we set u(x) = 0 outside G. Note that (e) does
not depend on u.
(34) The dual space. Let I < p, q < 00 and p-l + q-l = I. Then
(Lp(G)). = L 4 (G).
This relation is to be understood in the following sense:
(i) Let u E Lq(G) and set
U(v) = fG uvdx
for all v E Lp(G).
Then U: Lp(G) --+ R is a linear continuous functional on L,(G), i.e., U E
(Lp(G)).. Moreover, we have
IIUII = lIuli p .
(ii) Conversely, each U E (L,(G)). can be obtained in this way, where u E
Lq(G) is uniquely determined by U.
Consequently, there exists a normisomorphism u  U from Lq(G) onto
(L,(G))..
Identifying U with u, we may write
(u, v) = fG uvdx for all u E Lq(G), () E Lp(G).
(34a) The con vergence u" ..... u in L p ( G) as n ..... 00 means II u" - u II p ..... 0, i.e.,
fG lu" - ul P dx -+ 0 as n -+ 00.
(34b) The weak convergence u"--:a.u in Lp(G) as n -t 00 means (v,u">-+
< v, u> for all v E Lq( G), i.e.,
fG U"V dx -+ fG UV dx
as n --+ 00
for all v E Lq( G).
(35) A convergence theorem. Let I < p, q < 00 and p-l + q-l = I. From
u" -+ u in L,(G)
V n --:a. V in Lq{ G)
as n -+ 00,
as n..... 00,
it follows that
f. u"v" dx -+ f. uv dx
c; G
as n -t 00.
(36) Converge,zt subsequences. Let I  p < 00.

Lebesgue Spaces
1023
(36a) From
Ii" -. u in L,,(G) as n -+ 
it follows that there exists a subsequence (u".) such that
un.(x) --. u(x) as n .-. 00 for almost all x e G.
Moreover, there exists a function v E Lp(G) such that
lu,,#(x)1 < vex) for all n' and almost all x e G.
(36b) From the weak convergence
u,,u in Lp(G)
as noo
and
u..(x) ..... vex) as" .... 00 for almost all x E G,
it follows that u(x) = vex) for almost all x e G.
(36c) Let 1 < p < 00. Then each bounded sequence (II,,) in Lp(G) has a
subsequence (u.*) with
Un'  u in L,(G) as n.... 00.
(36d) Each bounded sequence ('4,,) in La)(G) has a subsequence (u".) with
.
U",u in L(G)
as n -. 00,
i.e., for all VEL I (G)
f. u".vdx  f. uvdx
G G
as n -+ 00.
The definition of the space La)(G) can be found in (39) below.
(37) Surface lneasure and surface integral. Let G be a bounded region in R"',
r > 2, with a sufficiently smooth boundary, i.e., oG E Co. 1 in the sense of
Section 6.2.
For sufficiently regular subsets of aG one can introduce a classical surface
area by using classical surface integrals. In terms of general measure theory
this leads to a premeasure on aG. By the main theorem of general measure
theory (7Sf) below, this premeasure can be uniquely extended to a measure
which we call the surface measure on aG.
According to (78) below, to each measure p. there corresponds the notion
of a Lebesgue integral J f dJJ. Let f: oG -+ R be an integrable function with
respect to the surface measure on aG. Then the corresponding Lebesgue
integral is denoted by
f. f dO.
cG
(38) The Lebesgue splIce Lp(oG). Let I S p < 00 and let G be given as in

1024
Appendix
(37). We set
lIull"oe<; = (Ie<; lul" dO )'IP 0
Let Lp(oG) denote the set of all measurable functions
u: aG -. R with lIullp,"G < 00.
Here, the measurability of u refers to the surface measure.
Then Lp(oG) together with the norm U' tp,"G becomes a real B-space once
we identify any two functions which differ only on a set of surface measure
zero on iJG.
Let N = 1 and G = ]a,b[ with - < a < b < oc. We set
HuUp,cG = (lu(a)IP + lu(b)I P )IIP.
Let L,(oG) denote the set of all functions u: oG -. R. Then Lp(vG) together
with the norm 1I.1I"cG is a real B-space.
(39) Tire space L(G). Let G be a nonempty bounded open set in (RN, N > 1.
The number B is said to be an essential bound for the function II: G -+ R iff
lu(x)1 s B
for almost all x E G.
We set
111111«1 = inf{B: B is an essential bound for u}.
Let Lc£(G) denote the set of all measurable functions
u: G -+ R with 111.1100 < 00.
Then L(G) together with the norm U' 11«1 becomes a real a-space once we
identify any two functions which differ only on a set of N-dimensional measure
zero.
(39a) Embedding theorem. Let I  q  p S 00. Then the embedding
Lp(G)  L.(G)
is continuous.
(40) The dl.al space to L 1 (G). We have
(L 1 (G»* = L:o(G).
This is to be understood in the sense of (34) with p = 1 and q = 'YJ. In
particular.
(u, v) = IG IIvdx
for all u E La:.(G), VEL 1 (G).
The spaces L 1 (G) and LX)(G) are not reflexive. Hence the notion of weak.
convergence on L(G) becomes important.

SoboJev Spaces
1025
(4Oa) Weak convergence in L 1 (G). The weak convergence
V,,v in L.(G) as II -to 00
means (fI, V" > .... < u, v> for all u e LC()( G), i.e.,
fG uV"dx -+ fG uvdx as n -+ 00 for all u E L<J)(G).
(40b) Weak. convergence in L(G). The weak. convergence
rl,,u in L(G) as II -+ 00
means (Un' v) -. (fI,V) for all v e L.(G i.e..
fG u"vdx -+ fG ut,dx as n -+ 00
(4Oc) A conl'ergellce theorem. From
for all veL. (G).
.
U" -:. U in L( G)
v" .... v in L. (G)
as II -. 00,
as n -+ 00..
it follows that fG ""V II d. -to JG UV dx as n .... OC).
Sobolev Spaces
Let G be a bounded region in R'Y with N > 1. The Sobolev spaces
W:(G) and W:(G)
\vith 1  P  00 and k = 0, 1, . . . have been introduced in Section 21.2.
(41) W:(G) is separable for 1 :$; p < 00.
(42) W;(G) is uniformly convex and hence reflexive for I < p < rfJ.
(43) Density. Let 1  P < 00. Then Co(G) is dense in wpt(G).
Moreover. the set CX,(G) n W:(G) is dense in W:(G).
If the boundary oG is piecewise smooth,1 i.e., aG E co. 1 , then C(G) is dense
in W,"(G).
The set C6'(R N ) is dense in W:(R'), and hence
W,t«(RN) = w,t(IR'V).
(43a) The universal extension theorem. Let I  P  (Xi and let oG e Co. 1.
Then there exisl a linear continuous operator
E: Wpt(G) -+ W:(R'>
I For J'I - I. the condition "cG e CO. I ,. means that G is a bounded open intcrval.

1026
Appendix
with
(EII)(x) = U(X)
for almost all x e G.
In addition, the extension Ell of II is c outside G.
The operator E is universal, i.e., E is independent of p and k = 0, 1, . . . .
The same result holds if G is an open half-space in AN. In this case, the
operator E can be constructed in such a way that the boundedness of supp U
implies the boundedness of supp Eu.
(43b) Let 1 < p < 00 and k = 0, 1, ... . Let G be a nonempty open set in
R N , N > 1. Then there exists a linear continuous operator
Eo: W;(G).... W:(R N )
such that Eou = u on G and tlEoull = lIuli for all II e W:(G).
Further material on the extension of functions can be found in Problems
21.7 and 21.8.
(44a) The dual spaces Wpl:(G)* and W;(G)*, negative norms and distri-
butions. See (71) below.
(44b) Sobolev spaces and the Fourier transform. See (74) below.
The Sobolev Embedding Theorems
Let X s }: The embedding operator E: X -. Y assigns to each x E X the
corresponding element x E Y: The embedding X  Y is called continuous or
compact iff the operator E is continuous or compact, respectively.
(45) Main embedding theorem. Let G be a bounded region in RA\' with N  I
and piecewise smooth boundary, 1 i.e., cG e CO. I. Let
O < j<k, 1 sp,q< x>, O<a< 1.
We set
1 k-j
d= p - N ·
Then the following fundamental assertions hold.
(45a) The embeddings
W:(G) C W/(G)
and
W:(G) c W/(G)
are continuous for d  l/q and compact for d < l/q.
I For. -- J. the condition "iJG € CO- I .. means that G is a bounded open interval.

The Sobolev Embedding Theorems
1027
This statement for W:(G)  Wj(G) remains valid if the boundary of G is
arbitrary.
(45b) The embeddings
W:(G) £ CJ.S( G ) c CJ( G )
are compact for d + rx/N < O. In particular, the embedding
W:(G) s CJ( G )
is compact for d < O. Le.. k - j > N /p.
More precisely, this means that a function u e W,A(G) also belongs to Ci'''( G )
and Ci( G ) provided we change u on a suitable set of N-dimensional measure
zero.
(45c) Let G be a bounded region in AN, N  1. The embedding
W;(G) c '(G)
is continuous for I S q S P S 00 and k = 0, I, 2, . . . .
(4Sd) The embedding
W:(R N ) c C)(R"')
is continuous if d < O. i.e.. k - j > N fp and 1 < p < 00. Here, we equip Cj(RJ\')
\vith the usual norm lIull).oo (cr. Section 21.2).
(45e) The embedding
W:(R1\') C W/(R"')
is continuous if 0 j < k, 1  p, q < 00 and d S I/q,
(46a) Standard exaIJJple 1. Let 1  P < 00 and let G be a bounded region
in RI", JV > 1. Then the embeddings
L,(G) ::> W,I(G) :J W,2(G) :J '"
are conJpact. If cG E Co. t , then the embeddings
Lp(G) ::) W,I (G) :J W,2(G) :) ...
are also compact.
(46b) Standard example 2, Let G be a bounded region in AN with oG E Co. 1 .
Then the embeddings
WlCG) C Lq(G) and Wl(G) C Lq(G)
are compact if either
N = 1, 2
and
lq<oc;
or
N > 2 and I  q < 2N f(N - 2).
'[his result remains valid for "'2' (G) if the boundary of G is arbitrary.

1028
Appendix
In Part IV we will use the compactness of the embedding
W 2 1 (G) S L 4 (G)
in (R3 in order to give existence proofs for the Navier-Stokes equations.
(46c) Sta,ulard example 3. Let G be a bounded open interval in (RI. Then
the embeddings
Wl +J(G) s; CJ.( G ) s; CJ( G )
are compact for 0 <  < 1/2 andj = 0, I, ... .
The following special cases of (45) show that this situation in R I becomes
worse for increasing dimension N.
(47) Critical exponents and prototypes of the general Sobolev embedding
theorems. We define the following critical exponents:
q crit =
pN for N > p,
--
N-p
00 for N < p,
N for N < p,
1--
p
-oc, for N > p.
crit =
Obviously, qcrU > p. Let G be given as in (45).
Case t: N < p < 00. The embeddings
W,I(G) c CZ( G ) c C( G), 0 <  < crl"
W,I(G) s Lq(G), I S q < 00,
are compact. Here, qCfU == 00. The embeddings
W p l (G) S; C2( G ), 1% = rtcril'
are continuous.
Case 2: I  p < N. The embeddings
W p 1 (G) S Lq(G)
are compact for 1 S q < qcrit and continuous for q = qcri'.
Case 3: p = N. The embeddings
Wi (G) s Lq(G)t 1 :s; q < 00,
are compact. Here, qcrit = 00.
Case 4: The embeddings
CX(G)  C'(G)  C(Gh
Co. le G) c C'( G ) c C( G 
o < II < 2 S 1,
o < p < I.
are compact.

The Sobolc\' Embedding Theorems
1029
Table I
p=2 p=3
dimension N qcrU I%C"t qait (XcIiI
I 00 li2 :tJ 2/3
2 ro -00 00 1/3
3 6 -X) 00 -ex:,.
4 4 -X) 12 -00
Case 5: N = I and I  p < co. Let G = ]a,b[ with -00 < a < b < 00.
Then the space W p 1 (G) consists of all absolutely continuous functions
u: [a, b] ..... R whose derivative u' (which exists almost everywhere) belongs
to L,(G). More precisely, each II e Wp.(G) has this property after changing
the values on a set of measure zero. The embeddings
W,l(G) c C.- I /P(G) s C(G), 1  p < 00,
are continuous.
Table 1 contains the critical exponents for p = 2, 3. Note that c'iC = -00
means that the favorable Case 1 is not at hand.
The general embedding theorems (45) follow from these prototypes.
(47a) Example. Let N = 3. By Case 2, the embeddings
W 2 Z (G) 5 I(G), 1 S q S 6,
are continuous. By Case 1, the embeddings
W 6 1 (G) s C 2 (G) 5 C( G ),
o < :x < 1/2,
are compact. Thus, the embeddings
W 2 2 (G) 5 C«( G )  C(G), 0 <  < 1/2,
arc compact. The same result follows from (45 b). Moreover, by Case 1, the
embeddings
w;l(G)  W 6 1 (G) s; C1jZ( G )
are continuous.
(48) Generalized bOllndar}' t'alues. Let G be a bounded region in R N with
N  1 and iJG e CO. I. Let 1 < p < 00. Then, for each such p, there exists
exactly one linear continuous operator
Bp: Wpl(G)..... Lp(oG)
such that, for all u E CI( G  the following holds:
Bpu = classical boundar}' function of u \vith respect to oG.
Ifu E W,l(G), then we call b = Bpu the generalized boundary function ofu (or
the trace of u).
The function b is uniquely determined as an element of the space Lp(oG).

1030
Appendix
That is, for N  2, b is uniquely determined up to changing the values on a
set of surface measure zero.
In the case where N = I, the embedding Wp'( G) S C(G) is continuous. That
is, the function u e W p 1 (G) is continuous on G = [a,b] after changing the
\'alues on a set of measure zero on G. Then B,u corresponds to the classical
boundary values u(a) and u(b) of this uniquely determined continuous function.
(48a) For all U E W,l(G),
II Bpu II L,,(.  const 11 u II w (G)'
(48b) U E W,I(G) is equivalent to
u e W p l (G) and u = 0 on oG
in the sense of generalized boundary values.
(48c) If u e Wp'(G), k > I, then
D'u E W,I(G) for all «: 10:1 < k - I.
This implies D«u = 0 on iJG for all ex: lexl S k - I, in the sense of generalized
boundary values.
(49) C/,aracterizat;on of generalized bou"dar}' valrles. Let G be a bounded
region in R N with N > 2 and oG e Co. t , and let I < p < 00. It is remarkable
that there are functions b e L,(oG) which are not the generalized boundary
functions to a function u e Wpl(G). Those boundary functions b E Lp(cG
which correspond to functions u E W"I(G), form the proper subset W p 1 - 1 IP(c1G)
of L,(oG). This underlines the importance of the Sobolev spaces W: of frac-
tional order k \vhich will be introduced in (500) below. More precisely, we
have the following two results which are very important for the modern theory
of boundary value problems.
(49a) For the boundary operator, the mapping
B,,: Wp'(G)-. W"t-lI'(oG)
is linear, continuous, and surjective.
(49b) There exists a linear continuous operator
E,: W"I-I/"(oG) -+ W,I(G)
such that the following diagram is commutative:
W,J(G) B.. W,a-I/'(oG)
 /.
W p t (G).
Consequently, exactly the functions
b e W p 1 - I /'(BG)

Sobolcy Spaces ot Fractional Order
1031
arc generalized boundary functions of functions u e W p l (G), and the function
u = E"b is an extension of the boundary function b to the region G.
Sobolev Spaces of Fractional Order
(SO) The space W:(G) for 'Ion;nteger k. Let G be a bounded region in R N
with N > 1 and oG E co. 1. Moreover, let 1 < p < 00 and
k = m +  m = 0, I, . . . , 0 < p. < I.
We set
f(u) = J. lu(x) - u(}'W dx d '
G xG Ix - YI'"#+pp )
and
( ) 1/'
II ull i ., = lIuH:'. p + L f(D.") ·
I S IJI
By definition,
" e Wp'(G) iff u e W;'(G) and lIullk.p < 00.
Then W;(G) together with the norm II-Ill.., becomes a real separable B-space
which is renexive for I < p < 00. The embedding
C""'( G )  W;(G)
is continuous for 0 < p < fl  I.
According to the definition above, the space W:(G) can be regarded as an
integral variant of the Holder space C"""( G ).
(5 1 a) rhe boundary space (oG) for 0 < k < 1. Let G be a bounded region
in R'v with N > 2 and aG e C .1 and let I  p < XI. We set
f(b) = J. Ib(x)_ - b(Y)I" dO dO
VGx2G Ix - )tl,Y-1 +,A
and
IIbllw:(r.Gt = (fG IblPdO + f(b)Y''',
By definition,
be W;(oG) iff be L,,(oG) and IIbllw:<cG) < oc.
Then W p l:(t3G) together with the norm 11.11 WJc(CG) becomes a real separable
B-space which is reflexive for I < p < 00. p
(5 I b) The boulJdar}' space W:(G) for noninleger k > I. Let
k = m +  m = I.  ..., 0 < It < I.

1032
Appendix
and let G be a bounded region in R N with N  2 and aG E C-. I. According
to Section 6.2, the boundary aG can be covered by finitely many Cm.l-surfaces
Si- Furthermore, with respect to a suitable local coordinate system, the surface
S, is given by the equation
, = '(),
 e C I ,
where ,: C; -t R is a Cm-I.function on the open (N - I)-dimensional cube C;.
We set
( ) J/P
IIbli W;(f 1 G) =  IIbU";(c" ·
By definition,
b E W;(cG) iff b E Lp(cG) and IIbllw;(i'G) < 00.
This definition includes tacitly that b e W;(C,) for all i.
Then W;(cG) together with the norm II-II wlcci'G) becomes a real separable
B-space which is reflexive for I < p < 00. It
Equivalent Norms on Sobolev Spaces
Let X be a B-space_ Rccall that the two norms II-Ill and 11-11 2 are equivalent
on X iff there are positive constants c and d such that
c lIull2 S lIull l S dllull2 for all u e X.
(52) A general theorem. Let G be a bounded region in R N ",'ith N  I and
oG E CO_I, and let I  p < 00, k = I, 2, ... . We set
(f. J ) IIP
lIuli = L IDClful" dx + L Jj(u'f ·
G IJj-i j-I
Rccall that
Ilu 1l1e., = (f. L I D2 U l P dX ) l/P.
G I  A:
Then the norms 11.11 and II. lit., are equivalent on the Sobolev space Wpt(G)
provided the following two conditions are satisfied:
(i) The functions Jj: W:(G) ...... Rare seminorms on W:(G) for allj with
o < .I)(u) < const IIIIIIJc.p for all II e W,.(G) and all j.
(ii) If
fP) = 0, j = I, ..., J,
for a polynomial P: Rl\' ... R of degree s; k - 1, then P = 0_
(53a) Standard eXII"Jple I. On the Sobolev space W p l (G), each of the follow-

The Gagliardo - Nirenberg Inequalities
1033
iog three norms is equivalent to the original norm Ilull!.,:
(f. N f. P ) I/p
.L ID;ul'dx + udx ,
G .=1 G
(f. N f. P ) IIp
.LID;uIPdx+ udO ,
G · = I ('G
(f. .f I Dju I P dx + f. I u IP dO ) liP.
G . = 1 ('G
Note that in the case where N = 1 and G = ]a, h[, -oc, < a < b < 'XI, we set
f. g dO = g(a) + g(b),
c'G
in contrast to our convention in Chapter 18.
Proof. This is a simple consequence of (52). Note that in this special case,
condition (ii) abol'e must be checked only/or P = constant. D
(53b) Standard example 2. On the Sobolev space W,k(G), the norm
Ilull k . p . O = (f. L ID2 U l P dx ) I/'
c; lal =t
is equivalent to the original norm /I.II.. p . This theorem remains valid if the
assumption i'G E Co.. drops out.
(53c) Standard e.xan,ple 3. Let G be a bounded region in R N , N > 2, with
(1G E Co. I. Let c. G and c 2 G be disjoint open subsets of cG with
-- --..
iG = (1. G U 02G,
VI G ¥- 0.
Then
(f. .f IDjul P dx + f. lulP dO ) I!P
G .=. raG
is an equivalent norm on Wpl(G), I S; p < 00.
Let X = {u E Wpl(G): u = 0 on 0 1 G}. Then
(f. N ) I/P
G j IDjulPdx
is an equivalent norm on X.
This fact is fundamental for the investigation of mixed boundary value
problems.
The Gagliardo- Nirenberg Inequalities
The following results arc fundamental for handling the nonlinearities of partial
differential equations by means of Sobolev spaces.

1034
Appendix
(54a) Let G = RAv, ti  I, or let G be a bounded region in R' with oG e
Co. 1. Let In = I, 2, ... and I S p, q, r S 00. The for all
u e W,"'(G) () L 4 (G),
we have
IID'u II, s const null.p lIull-e
provided that 0  IPI "I - 1,6 = IPI/m, and
! = IIlI + 6 (  _ m ) + (1 - O)!.
r N p N q
If m - 1111 - N fp is not a nonnegative integer, then the values IPI/ln  9  1
arc allowed for 6.
(54b) If G = (liN, then
IID-flar s const Cr... II l)'Zfl U" r lIull -',
for all u E W,"'(G) () L.(G).
(54c) Banach algebra. We set X = Wp"'(G where m = 1, 2, ... and 1 < p <
00. Let G = Rt.. or let G be a bounded region in R' with N  I and oG e CO- J.
Furthermore, let
mp > N.
Then the Sobolev space X forms a generalized Banach algebra. i.e., u, veX
implies uv e X and lIuvll < const II f41111vll for all u, veX.
This result remains true for m = 0 and p = 00.
(55) Abstract interpolation operator on Sobolev spaces. Let Q be an open
cuboid or an open simplex in R N , N  1, where
h = diameter of Q;
p = radius of the largest ball contained in Q.
We set
(Au)(x) = u(Bx + b),
where B: RN -.. AN is an arbitrary linear bijective operator, and b is an
arbitrary clement in DiN. Furthermore, let I  p  00 and 0  m  k + I,
where m and k are integers.
Suppose that the linear continuous operator
n: W,t+l(Q) -. W,"'(Q)
has the following two properties:
(i) nu = u for all polynomials of degree S k.
(ii) n is affinely invariant, i.e., we have nA = An for all the operators A
introduced above.

Galerkln Schemes in Sobolev Spaces via Polynomials
1035
Then, for all U E W p " + I (Q),
(55a) lIu - nul/",.p.o < const h"'" p-'"lI u ll,+1.p.o.
The constant depends on N, k, m, and p, but not on Q.
This result will be used basically in (59) below in order to prove important
approximation properties of finite elements. The proof proceeds analogously
to the proof of Proposition 21.52 (cf. Ciarlet (1977, M), p. 121).
Galerkin Schemes in Sobolev Spaces via Polynomials
(56) Polynomial basis in Wp"(G). We set
"'i I ... i.rw (x) =  .  l . . .   ,
where ii' . . . , iN = 0, I, . . . , x = (1'. . . 'N). Let G be a bounded region in R N
with cG E co. I and N > 1. Furthermore, let I < P < 00 and k = 0, 1,2, ... .
Then all the functions w. form a basis in Wp'(G). If we set
X" = span{w il ... iN : 0 < ;1 + ... + iN < n},
then X" consists of all real polynomials of degree < n, and (X,,) forms a
Galerkin scheme in Wp"(G).
(57) Quasi-polynomial basis in W:(G). We replace the functions "'.. above
by
(1. . ( x ) = cp( x ) " w. . ( x )
'. I. I. . 'N '
where ii' . . . ,( = 0, I,. . . . Let G and G I be bounded regions in [RN with G c G.
and N > I. Furthermore, let I < p < 00 and k = 0, I, ....
Let cp: G I  IR be a fixed function with cP E C k + 2 (G) and
cp(x) = 0
iff
X E G.
Moreover, assume that
N ( tJCP{X} ) 2
L ¥O
i = I a i
We set X = Wp"(G) and
X" = spa n { l'i. ... iN: 0  i l ,. . . , iN < n}.
for all x E aGo
For fi x ed r = 0, I, . . . , }' E [0, I] and k = I, 2, . . . , let
y = {u E c"+r'Y(G): DCl U = 0 on iJG for all a: lal < k - I}.
Then:
(i) All the functions v.. form a basis in X, and (X n ) is a Galerkin scheme in X.
(ii) For all u E Y,
. J const
dlstx(u, An)  + lIuJl y ,
n r Y
n = I, 2, . . . .

1036
Appendix
The constant depends on k, r. I. p, and G. Cf. Ciarlet, Schultz, and Varga
( 1969).
Galerkin Schemes in Sobolev Spaces and
the Method of Finite Elements
(58) Basic ideas. Suppose we want to solve an elliptic boundary value
problem via the Galerkin method. If we use a polynomial Galerkin scheme
as described in (56) and (51) above, then, as a rule, the corresponding matrices
are not sparse matrices, i.e., most of the entries are different from zero. This is
a typical shortcoming of polynomial bases. In contrast to this unfavorable
situation, both the difference method and the method of finite elements
produce sparse matrices. Compared with the difference method, the method
of finite elements is much more flexible. For example, we can vary the mesh
size of the triangulation in different subregions depending on the expected
subtle behavior of the solution (Figs. 2(a), (b). Today, the method of finite
elements is widely used in engineering and in the natural sciences. This
method represents one of the important achievements of modern numerical
mathematics.
(58a) Prototype for finite elements in R 1 . Let (X < p < )'. We start with
{ o if x = a, }',
w(x) = 1
if . = p,
and extend \v to a function \"': IR .-. R via linear interpolation. This way
a
b
f ,  I
a
I ·
b
(a)
(b)
I
a
a,
b
Q P Y
(d)
(c)
Figure 2

Galerkln Schemes in Sobolev Spaces and the Method of Finite Elements
1037
we obtain
o
",(x) = (x - ex)/( P - ex)
(y - x)!(,' - IJ)
if x f [a, }'],
if ,x E [2, PJ.
if x E [P, y],
(Fig. 2(d)). We now consider a triangulation !Y of the compact interval [a. h],
i.e.. we choose nodes a; such that a = a o < a I < a 2 < ... < ale = b. The mesh
size of .'T is defined through
h(ff) = max (°.+ 1 - a;).
i
We set
\\i(a j ) = {
if ; = j,
if ; #: j,
and extend \\j to a function '''j: [a, b] -. R via linear interpolation. The func-
tions w o , "'1' . · · , "', are called finite elements. Furthermore, we set
X (:T) = spa n { "'0' · · . , 'Vie }
and
X(Y) = span{"'I...., W'_I}.
Then, X (Y) consists of all piecewise linear, continuous functions "': [a, b] -+ (Ji
with arbitrarily prescribed values I(ao)' .... "'(a,) at the nodes (Fig. 2(b)).
Moreover, we get
X(y) = {", E X(ff): '(a) = \\'(b) = OJ.
Obviously, we obtain the generalized orthogonality relations
J..b wj(x)wj(x)dx = 0 if Ii - jl > 2.
These relations are responsible for the appearance of sparse matrices in con-
nection with the Galerkin method.
Now, let () be a sequence of triangulations of the interval [a. h], where
the mesh size goes to zero as n -+ 00, i.e., 11(Y,.) -+ 0 as n  OC'. Let 1 < p < Ix:,
and set h ll = h(y") as well as XII = X(ff ll ) and XII = X(). It follows from
Section 21.14 that:
(a) (XII) forms a Galerkin scheme in X = W p l (a, b).
(b) For all U E W,2(a, b),
distx(u, XII)  const hllilull 2.p'
i.e., the accuracy of appro,ximation ;s proportional to the Inesh size h,..
(c) (X II) forms a Galerkin scheme in X = W p 1 (a, h).
(d) For all u E W p 2 (a,b) 11 X.
distx(u, XII)  const hlil/llII 2.p'
The constants in (b) and (d) depend on h - a and p.

1038
Appendix
P,
P l
P 1
(a)
(h)
Figure 3
(59) Piecewise linear finite elements in (R2. We want to generalize the
previous results from Al to R 2 . Let G be a bounded polygonal region in A 2 .
We choose a triangulation :T of G with nodes N 1 ,..., N" (Fig. 3(b)). Moreover,
we set
h(ff) = maximal diameter of the triangles of ,,
9(ff) = minimal angle of the triangles of ff.
The following elementary result is decisive for the construction of finite
elements.
(L) Let T be a triangle with vertices PI' P 2 , PJ. Then there exists a unique
linear function w(,,,) = a + b + c" with prescribed values w(P I ), w(P 2 ),
w(PJ ).
Construction (C). We now prescribe the values w(N I ), . . . , w(N,,) at the nodes
and extend w uniquely to a function \v: G --+ R via linear interpolation accord-
ing to (L). Then w has the following properties.
(i) \v is continuous on G.
(ii) w;s piecewise differentiable, and W E W p 1 (G), I  p  00.
Proof Ad(i). Let TI and T 2 be neiboring triangles with the joint side PI P2.
Then w is uniquely determined on PI P 2 by the values at the points PI and P 2 .
Ad(ii). This follows from Example 21.6. 0
The set of all the functions w constructed by (C) above is denoted by X(ff).
In particular, if we set
wj(N j ) = {
if i = j,
if i:F j,
and if we construct Wj: G --+ R by (C), then the so-called finite elements w J form
a basis of the space X (ff), i.e.,
X (ff) = spa n { WI' . . . , w" } .
Furthermore, we set
X(ff) = {w E X(.): w = 0 on oG}.
Obviously, X(Y) consists of all W E X(ff) with w(N i ) = o for all nodes Nt E G.

Galcrkin Schemes in Sobolcy Spaces and the Method of Finite Elements
1039
If the nodes N i and  do not belong to neighboring triangles, then
(w,l"j)'.2 = f. ( WIWJ + f DrWiDr"J ) dX = o.
G ,-1
This generalized orthogonality relation produces sparse naatrices in connec-
tion with the Galerkin method.
Finally, we consider a sequence (5;,) of triangulations of G, where the mesh
size goes to zero as n -+ oo i.e.,
lim h(5;,) = o.
"  r.o
Moreover, we assume that (9;,) is nondegenerated, i.c. the minimal angles of
the triangles are bounded below:
inf 3" > O.
"
We set hIt = h(9;.) as well as XII = X(9;,) and i. = X($;.). Let 1 < p < 00.
Then the following hold:
(a) (X,,) is a Galerkin scheme in X = W p 1 (G).
(b) For all u e W p 2 (G),
distx(u, X.) S const h"lIuIl2.p.
(c) (X,,) is a Galerkin scheme in X = W,I(G).
(d) For all u e W,2(G) r\ i,
distx(u, i,,) s const h.1I ull".".
The constanls in (b) and (d) depend only OIl G, p, alJd inf./J.".
Proof. We will use theorem (55) on abstract interpolation operators.
Ad(a), (b).
(I) Let T be a triangle of the fixed triangulation 9;. of G. For m = 0, 1, we
construct the operator
n: W,1(G) -. Wp.(G)
in the following way. Let u e W,2(G) be given. This implies u e C( G ) by
the Sobolev embedding theorems. Thus it is meaningful to set
(nu)(N,) = u(N,)
and to extend this to a function nu: G -. R via linear interpolation
according to (L) above. Note the following:
(IX) The construction of nu is unique by (L), and we have nu e W,IIt(G) n
X" by (ii) above.
(p) nu = u on the triangle T if u is a linear function on T.
(i') n is affinely invariant on T in the sense of (55).
Consequently, we can apply theorem (55) to the triangle T. For m = O.
1, this yields
lIu - nuUf..I(T) s C(h; + h/p.)"Uullfvl4T)'
, ,

1040
Appendix
where C denotes a constant. Summing over all the triangles Te 5;., we
find
1111 - null'r'(G) < C(h; + 1a;/Pn),lIull2(Gt.
p p
(II) We now consider the sequence (9;.) of triangulations. We set "n = n.
From inf" 8n > 0 we obtain the key relation
sup lJ"jp" < 00.
n
Thus, we get
lIu - n"f 4 I1wl(G) < const 1J.llullwlcGt.
p p
Since nllu e X n , we obtain (b), i.e..
dist.t(u, X n )  const h n llu8 w:«(;)'
(III) Density. Let 14 e W,I(G) be given. The_set (G) is dense in W"I(G). Thus.
for each € > 0, there exists a v e CXI(G) with lIu - vlll,p < £. This implies
1111 - nnt'llt., S lIu - vi! I.p + IIv - n.vIl 1 ."
S I: + const h.1I vII ZIP S 2£
for all II > no(t). Since "..veX,.,
lim distx(u, X n ) = O.
"aJ
Therefore, (X,,) is a Galerkin scheme in W"I(G).
Ad(c), (d). This follows similarly to (a), (b).
o
(59a) Generalizatioll to piecewise quadratic finite elenaents. The previous
results can be easily extended to piecewise polynomial functions of degree
k > 2. To explain this we consider the special case k = 2.
Let T be a trianglc with vertices PI' P 2 , P 3 . Denote by P4' Ps, P 6 the
midpoints of the sides of T (Fig. 4). The following elementary result will be
crucial.
(Q) There exists a unique quadratic polynomial
",(,,,) = a + b + ell + d2 + e,,2 + "
\vith prescribed values w(P I ). . . . , W(P6).
P;
PI
P!t
P2
Figure 4

Galcrkin Schemes in Sobolev Spaces and the Method or Finite Elements
1041
Let . be a triangulation of G (Fig. 3 (b». For each triangle Te ff, we
prescribe the values \v(P I ), . . ., "7(P 6 ). Using (Q), we extend these values to a
function \\': G ..... R. Then '" has the following properties:
(i) \\1 is continuous on G.
(ii) '\-' is piecewise differentiable, and \\1 e W,l(G), 1 < p S 00.
This follows from Example 21.6. Let X(2)(ff) denote the set of all these
piecey,'ise quadratic polynomials. Moreover. we set
X(2)(ff) = {", e X (2 )(T): \\1 = 0 on oG}.
As in (59) above, \ve now consider a sequence () of triangulations with h n -. 0
as n -+ 00 and inf" 8" > o. let 1 S P < 00. Set Y" = X(2)(9;.) and  = (2)().
Then the following hold:
(a) (f,,) is a Galerkin scIJenle ill X = Wpl(G).
(b) For all u e W p 3(G),
distx(u, )  const h; lIuIl3.p.
(c) () is a Galerkill scheme in X = W p l(G).
(d) For all u e W;(G) tI X,
distx(u, Y,,)  const h; !lr4[) 3.p.
Tile cOIJStants in (b) and (d) depend on/}' OIl G, p, and inf" 8".
Note that, in contrast to the piece\vise linear finite elements in (59) above,
\ve find here a higher-order accuracy of the approximation according to the
appearance of II;. Roughly speaking, it follows from our proof below that we
have the following situation in the case where 0  m  k + 1:
III the Sobolev space W;(G), the accr4raC}' o.f approximation of W;+I(G)-
junctions by piece,,'ise po/}'nonlial functions of degree k is proportional to
hie" 1 -"',
"'here h denotes the mesh size of the triangulation.
This result remains true for all bounded polyhedral regions in [R!', J\r > 1,
provided (k + l)p > Nand 1 S P < 00.
Proof. We prove (a)-(d). Let k = 2 and m = 0, 1. We will use an analogous
argument as in (59) above. The key to our proof is to construct the operator
n: W,A+I(G) -+ W"JII(G)
by setting
(nu)() = u(P i ),
i = 1,..., 6,
on each triangle Te ff", and by extending these values to a quadratic poly-
nomial on T via (Q) above. The point is that:
nu = u on T provided u is a polynomial of degree S k.

1042
Appendix
Hence, it follows from (55a) that
Ilu - nUIlW(T) S; C(h+1 + h:+ 1 /p..)lIullk+l.p'
This implies the assertions (a) -(d) as in the proof in (59) above.
o
(60) Piecewise polynomial functions on cubic grids. Let G be a bounded
region in R N with oG E Co. 1 and N > I. Furthermore, let m = 0, I, ... and
1  p < 00. Our goal is to construct systematically finite elements in the
Sobolev space W,"'(G).
(60a) Special finite elements in R I. We start with the function
7[1 (x) = {
if 0 < x < 1 t
otherwise,
and define inductively
7t m + t = 1t 1 .1t""
m = I, 2, . . . ,
where f . g denotes the convolution, i.e.,
(f. g)(x) = r: I(x - y)g(y)dy"
Figure 5 shows 7r I' 7r 2' 7r J' Explicitly,
n 2 (x) = 2 - x
o otherwise,
x
if x E [0, I],
if x E [I, 2],
and
nJ(x) =
x 2 j2
- x 2 + 3x - 3/2
(x 2 - 6. + 9)/2
o
if x E [0, 1],
if x E [I, 2],
if x E [2, 3],
otherwise.
Generally, we have
m (x - k)J k ( m + I ) (k - i)'"-j
7[11I+ I (x) = L "' L (- 1)' " (_ ")'
)=0 J. i=O , m J.
RI
I
J.
.
nJ
I
(a)
I 2
(b)
2
(c)
3
""igure 5

Ordinary Differential Fuations and Measurablc Functions
1043
for all x E [k, k + 1] with k = 0, 1, . . . . m and
7t m + 1 (x) = 0 for all x, [O,m + 1].
Here, we agree to set "0 0 = 1.'" For In  1, these functions have the following
properties:
(i) 1l",+ I has continuous (m - 1 )th derivatives on Rand piece\\'ise continuous
nJth derivatives on R, i.e., 1t..+l e C",-I (Ui)" W,,"'(R).
(ii) J 1l",. I (x) dx = 1.
(60b) Cubic grids in AN. We consider a cubic grid in RN of width h, i.e., the
grid points are given by
)' = (m I h, · . . , m.v h),
where ma, ..., nlN are arbitrary integers. Set .1C = (I"'" N) and define
Fm+.(x) = J] 7[.+1 (  ).
Furthermore, we define the finite elelnenls F+, : G -. R by
F+, (x) = F"'+I (x - y),
where )' denotes an arbitrary grid point. Finally, we set
X" = span{F+,: y = grid point}
and
x" = {F EX,.: suppF c G}.
Then the following hold, where n'lIi, 2 refers to W 2 Jt (G).
(i) For all U E W 2 "'+I(G) and 0 < s S nl,
inf Nu - vIIs.:! S const h",t l -"'n"lIm+l.2-
Co' eX"
(ii) For all u E W 2 111 + 1 (G) " Wi'(G) and 0  sSm,
in! II u - Vil".2 S const h",+I-'1I ulI.+ 1. 2'
p x"
(iii) If (hit) is a sequence with h n -. 0 as n  co, then
(X....) (resp_ (X",,»
forms a Galerkin sclrenre in WplJl(G) (resp. Wp"'(G».
The proof can be found in Aubin (1972, M).
Ordinary Differential Equations and Measurable Functions
For given Xo e R''I and to e A, we consider the initial value problem
(P)
X'(l) = 1(1, x(t»,
x(t o ) = Xo
t E U,

1044
Appendix
along with the integral equation
(P*) X(l) = Xo + i ' f(s. x(s» ds.
'0
t e U.
Let U c J and set
J = {I e R: II - '01 s ro}, K = {x e AN: Ix - .ol  T},
where T, To > 0 and N > 1. Letting x = (1'...' 1l) and I = (/1'... ,IN)' prob-
lem (P) can be written in the following form:
i(t) = /;(1, :«t)),
i(tO) = 'o.
;= I,...,N,
t e U,
(61) Theorem of Caratheodor}'. We assume:
(i) The function f: J x K -+ R satisfies the CaratIJeodor)' conditiolJ, i.e., for
all ;,
1.-. fi(t, x) is measurable on J for each x E K;
_'"  /,(1, x) is continuous on K for almost all t E J.
(ii) ,Wajorallt. There exists an integrable function J\l: J --. R such that
1J;(I, x)1 S M(t)
for all (I, x) E J x K and all ;.
Then:
(a) There exists an open neighborhood U of to and a continuous function
x( .): U -+ R N ,
which solves the integral equation (P*).
(b) For almost all t e U, the derivative _,"'(I) exists and (P) holds.
(c) The components  1 ( .), ..., N(.) of x(.) have generalized derivatives on
U, and x(.) is a solution of(P) on U in the sense of generalized derivatives.
This result remains true if J is a one-sided neighborhood of to, i.e., J =
{I e A: 0  t - to S 'oJ. Then U = {t E J: 0 S t - to < 'I}' where '. > o.
If the assumptions (i), (ii) are satisfied for K = R.". then U = J.
The proofs can be found in Coddington and Levinson (1955. M), p. 43, and
in Kamke (1960, M), p. 193.
Distributions with Values in B-Spaces
Bet\\'een 1930 and 1940, several mathematicians began to investigate systemati-
cally the concept of a Uy,'eak" solution of a linear partial differential equation.
which appeared episodically (and without a name) in Poincare"s work... .
It was one of the main contributions of Laurent Schwartz when he saw, in
1945, that the concept of distribution introduced by Sobole\' (which he had

Distributions with Values in B-Spaces
1045
rediscovered independent)') could gi\'e a satisfactory generalization of the
Fourier transform including all the preceding ones. . . .
To the credit of Laurent Sch\\'artz (born in 1915) must be added his persistent
efforts to weld all the previous ideas into a unified and complete theory, \\'hich
he enriched by many definitions and results (such as those concerning the tensor
product and the convolution of distributions) in his now classical treatise
(Schwartz., 1950). By his oy,'n research and those of his numerous students, he
began to explore the potentialities of distributions. and graduall)' succeeded in
convincing the world of analysts that this new concept should become central
in alllincar problems of analysis, due to the greater freedom and generality it
allowed in the fundamental operations of calculus, doing away with a great many
unnecessary restrictions and pathology.
The role of Schy,'artz in the theory of distributions is very similar to the one
played b)' Ne\\.ton and leibniz in the history of calculus: contrary to popular
belief, they of course did not invent it, for derivation and integration were
practised by men such as Cavalieri, Fermat, and Roberva) when Newton and
Lcibniz were mere schoolbo)'s. But they were able to systematize the algorithms
and notations of calculus in such a way that it became the versatile and po\\'erful
tool which we know, whereas before them it could only be handled via com-
plicated arguments and diagrams.
Jean Dieudonne (1981)
Distributions generalize classical functions. In contrast to classical functions,
distributions possess derivatives of arbitrary order. This crucial property of
distributions is responsible for the fact that the modern theory of linear partial
differential equations is based on the theory of distributions. The most impor-
tant notions of the theory of distributions are:
derivative, tensor produc and convolution of distributions;
Fourier transform of distributions.
OUf main applications to linear partial differential equations concern:
the fundamental solution,
the construction of solutions via convolution and fundamental solution,
and
the generalized initial value problem.
In the following let G be a nonempt}' open set in A,I with N  1, and let
0( = A, C. For brevity, we set
(F)
£!)(G) = Co(G).
The relation between classical functions u and distributions U is based on
the following simple formula:
U((j) = fa Il(x)(j)(x)dx
for all cp E f2( G).
However, note that there are distributions which cannot be represented by (F).
The basic strateg}' of the development of the theory of distributions consists
in formulating properties of functions u in terms of distributions U via (F).

1046
Appendix
This strategy ensures that distributions can be regarded as generalized func-
tions. For example, if the function u has the classical derivative v = D2u, then
v corresponds to the distribution
V(rp) = JG DZu(x)rp(x)dx for aU rp E (G).
Integration by parts yields
JG Dllrl(x)rp(x)dx = (-1)'«1 JG u(x)1)IIrp(x)dx.
Hence
v (cp) = ( - 1 )121 U (1)11 cp ).
This leads us in a natural way to the definition of the derivative lY' U of U by
setting D U =  i.e.,
(D)
(D 2 U)(cp) = ( - 1)121 U(DClip)
for all ip e (G).
We shall show below that this definition is also meaningful for general distri-
butions which do not correspond to a function via (F).
In the same way we will translate the following notions for functions to
distributions: the tensor product, the convolution, and the Fourier transform.
(62) Introductory example: the Dirac delta function. Since the 1930.s,
physicists use the so-caUed "c5-function;. which describes the density of a mass
point with mass m = 1 at Xo. According to this physical interpretation,
physicists set
b(X - xo) = { o
+00
if X:F Xo,
if x = XOt
and
(62a)
f. 6(x -xo)(x)dx = (xo)
R".
for all cp E 9(G).
In terms of mathematics, such a definition is nonsense because there is no
function having these two properties. However, the intuition of physicists led
them to an important new mathematical tool. The rigorous definition of  will
be given in (64a) below.
(63) Conl,ergence in the space (G). Denote by (G, K) the set of all err-.
functions
14: G -+ K
having compact support, i.e., there is a compact subset K of G such that u = 0
outside K. According to our earlier definition, 9}(G, IR) = f2(G) = C6(G).
Let (ip.) be a sequence in (G.IK) and let tp E (G, K). By definition, we write
CPII -+ cP in (G, K) as n -+ 00

Distributions wilh \'alues in B.Spaccs
1047
iff the following hold:
(i) For aU a with lexl  0, we have the uniform convergence
Dtl.cp" ::; Dtl.cp on G
as '1 ...... 00.
(ii) There exists a compact subset K of G such that </'11 = tp outside K for all n.
(64) Definition of distributions. Let Y be a B-space over k. By a distribution,
we understand a linear, sequentially continuous map
(M)
U: (G, 1<) -. Y.
i.e.. U is linear and, as n  00,
(/J,. -. ep in 9}(G, K) implies V(ep.) -. U(Q?) in Y.
The set of these distributions is denoted by
'(G. Y).
In the case where Y =  we briefly write '(G). In (72) below we will introduce
a topology on (G). In the sense of this topology, distributions are linear
cont;lJuous maps of the form (M). Thus, '(G) is the dual space to (G).
(64a) Standard example: the delta distribution. For fixed Xo E R rt ., we set
bxo(tp) = lp(xo) for all cp e fi(R N , K).
This definition is based on the idea (62a) of physicists. Obviously, 6"0 is a
distribution, i.e., b Xo e '(G, K). This distribution is called the Dirac delta
distribution at Xo. We set b = b if Xo = o.
(65) Classical functions and regular distributions. Let u e L..aoc(G, Y), i.e..
the function
u: G ... Y
is integrable on each compact subset of G. We set
(R) V(q» = fG u(x)q>(x)dx for all q> e !iJ(G.K).
Then U is a distribution, i.e., U e g}'(G, Y). Furthermore, let ve Li.ioc(G, Y)
and set
V(q» = fG v(x)cp(x)dx
for all tp E (G, 1<).
Then
(R.)
u=v
iff
u(x) = v(x)
for almost all x e G.
A distribution U is called regular iJTit can be represented in the form (R), where
u e L 1 . loc (G, Y). In the sense of (R.), there exists a linear bijective map from
L 1 . I0 4:(G, Y) onto the set of regular distributions in fJ'(G, Y). In this sense, we

1048
Appendix
can identry each function
U E L I.loc ( G, Y)
with a regular distribution, i.e., L1.loc:(G, Y) C !i}'(G, Y).
This justifies the designation "generalized functions.... for distributions. Note
that the delta distribution is not regular.
(65a) The support of a function. Let u: G -+ Y be a function. Recall that the
support of u is defined to be the set
supp u = closure of {x e G: u(x) =F O}.
(65b) The support of a distribution. Let U e '(G, Y) and let H be a non-
empty open subset of G. By definition,
U = 0 on H iff U(cp) = 0 for all cp E !?}(H, 1<).
The support of U is defined to be the set
supp U = {x E G: U =F 0 on each V(x)},
where V(x) denotes the intersection of G with an open neighborhood of ...
For example, if u E L 1.loc( G, Y) and if u is continuous, then
supp u = supp V,
where U denotes the regular distribution corresponding to the function u.
(65c) Standard example. For the delta distribution,
supp b Jto = {x o }.
(65d) Multiplication of a distribution by a COO-function. Let U E '(G, Y) and
let a: G --. I( be a COO-function. We set
(aV)(cp) = V(acp)
Obviously, aU E !?t'(G, Y).
for all cp E f!J(G, IK).
(66) Derivatives of distributions. Let U be a distribution, i.e., V € '(G, Y).
For each  with I(XI > 0, we define
(DCI U) ( <p) = ( - 1 )IClI U ( DCI cp )
for all cp e (G, K).
Obviously, D" U is again a distribution, i.e., D(J U e !?}'(G, Y). We call DCI U the
D".derivative of U. Consequently, we obtain the following decisive result:
Distributions possess derivatives of arbitrary order.
(66a) Standard example. For fixed Xo E R, consider the so-called Heaviside
function
u(x) = {
if Xo < x,
if x  X o -
Then, u' = b Jto in !?}' (R).

Distributions with Values in a-spaces
1049
Proof. Set
U(cp) = fA u(x)cp(x)dx for all cp E '@(IR).
By definition, V'(tp) = - V(tp') for all cp E ft(R). Hence
U'(cp) = - r2> cp'dx = cp(x o ).
J%O
i.e., U'(<p) = £5%0(<p). According to (65), we write u' instead of U'.
(66b) Example. Let
o
1 if .'(0 < x,
u(x) = -I if x > X o ,
arbitrary if . = x 0 .
Then, u' = 2b%O in ft'(IR).
Proof. For all tp E !l(IR), U'(<p) = - U(cp'), i.e.,
U'(cp) = f ::. cp dx - 1: cp dx = 2cp(x o ).
(66c) Example. For the delta distribution, we find
(Darb.lo)(cp) = (-1)1c11b.xo(Darcp) = (-I)larIDatp(xo).
for all cp E !t(R).
(66d) Generalized plane waves. We consider the wave equation
o
(W)
der I
Du = 2 u" - u = 0
c
for u = u(x, t), where .X E R 3 and t E R. Let n be a fixed unit vector and set
'IX = (nix). Then:
(i) If (,' E C 2 (R), then the function
(W*) u(., t) = v(nx - Cl)
is a classical solution of (W) on R 4 , which is called a plane wave.
(ii) If l' E L:.(joloc(R), then u in (W*) is a solution of (W) in the sense of distri-
butions in !i"(R 4 ).
By (W*), the discontinuities of u propagate along the moving planes
nx - ct = const.
Recall that v E LCX'o'oc(lR) iff the function v: R -+ R is measurable and bounded
on each compact subset of R, e.g., v is piecewise continuous.
This result shows that the theory of distributions is able to describe reason-
able physical situations, which lack regularity.

1050
Appendix
Proof. Ad(i). This follows from a simple computation.
Ad(ii). For all cp E (R4), set
U (cp) = r u(x, l)cp(X, I) dx dl.
JR.
We have to show that (l/c 2 )U,,(cp) - (U)(cp) = 0, i.e.,
U ( c 12 cp" - Acp ) = 0
for all cp E gJ(R 4 ).
That means
J dcr r v(nx - cI)Dcp(x,l)dxdl = 0
JR.
for all cp E (1R4).
To show this fix cp E !?}(R 4 ). Since supp cp is compact, there exist an open ball
B and an open bounded interval  such that supp cp c Band
nx - ct E &f
for all (x, t) E B.
Since v E L 1 (£f), there exists a sequence (VA;) in !liJ(R) such that
Vi() -+ v() as k -. 00 for almost all  E [}I.
Moreover, there is a C > 0 such that
IVi()1  C
for all  E EM and all k.
This follows from the properties of the smoothing operator in Section 18.14.
Let
J" dcr is v,,(nx - CI) Dcp(x, I) dx dl.
Integration by parts yields
J" = is cp(x,I)Dv,,(nx - ct)dxdl = 0,
since Dl',(nx - ct) = 0 by (i). Using majorized convergence A 2 (19), we obtain
J Ie -+ J
as k -+ 00.
Hence J = O.
o
(67) The tensor product of distributions.
(67a) Functions. Let u: R N -. IK and v: R M -+ IK be functions. We set
(u (8) v)(x, y) dcf u(x)v(y) for all (x, y) E AN X AM
and call the function u @ v: R N + M -. IK the tensor product of u and v.

DIstributions wIth Values In O-Spaces
1051
(67b) Regular distributions. Let u E L1.loc(IR N , IK) and v E L1.loc((R"', ).
Denote by U (8) V the regular distribution corresponding to II <8> v, i.e., for all
cP E !l'(IR N + M , IK),
(U@V)(qJ)= r (u@v)(x,Y)qJ(x,y)dxdy
JRItI...
= fRN u(x) (fHII V(Y)qJ(X,Y)dY)dX
= U(V(cp(x,.))).
(67c) General distribut;o,zs. Let U, V be given as in (67d) below. For all
CP E g(R N + M , IK), we set
(T) (U C8> V)(cp) dcr U( V(cp(x, . ))).
This is to be understood in the sense of
U ( V ( <P (x, . ))) = U (tjJ ),
where tjJ(.x) = V(cp(x, . )).
This way we obtain a distribution, i.e., U <8> V E ft"(IRN-+ M, IK). The distribution
U (8) V is called the tensor product of U and V.
(67d) Properties of the tensor product. Let
U E !t'(IR N , IK), V E q'((RM, IK),
(i) For all cp E £.t(IR N + M , IK),
(U @ V)(cp) = U(V(cp(x, .))) = V(U(cp(.,y))).
We g'(RL, IK).
This is briefly expressed by
UJC (8) , = V)' (8) U:x.
(ii) Let cp(.x, }') = a(.:()b(y), where a E !1'(R N , IK) and b E (RM, 8(). Then,
(U @ V)(cp) = U(a)V(h).
(iii) (U (8) V) @ w = U (8) (V (8) W).
(iv) D:(U @ V) = (DU) @ V for all lX: I(XI > o.
(v) D;( U (8) V) = U @ (D; V) for all lX: II > o.
(vi) supp U (8) V = supp U x supp V.
(68) Convolution of distributions. Let U, VE (IRN, IK) and suppose that
supp U is bounded. We set
(U.V)(<p) der (U@V)(cp.) forall <pE(RN,K),
where <P.(x, y) = cp(x + y). Then, U . V is a distribution, i.e., U . V E ft'(fRN, IK).
This distribution is called the convolution of U and V.
(68a) Regular distributions. Let u, VEL I.loc(R N , IK) and suppose that supp u

1052
Appendix
is bounded. For all x E (RN, we set
(u. v)(x) = r u(x - y)v(y)dy.
JRN
Then. u . VEL Itloc(R N , 0<).
Let U and V be the regular distribution corresponding to U and [', respec-
tively. Then, U . V is the regular distribution corresponding to u . ['.
(68b) Properties of the convolution. Let V,  We .@'(R N , 1\) and suppose
that supp V is bounded. Let A, JJ E IK. Then:
(i) Linearity: U . (A V + JJ W) = l( U . V) + JJ( U . W).
(ii) Commutativity:U. V = V. U.
(iii) Associativity: (U. V). W = u. ( V . W) if supp U and supp V are
bounded.
(iv) Derivative: Drl( U . V) = (D rl U). V = U . D rl V for all tX: ItXl  o.
(v) t5.W= W.b = W
Distributions and Linear Partial Differential Equations
(69) Funda,nental Solulion. We consider the linear differential equation with
constant coefficients
(E)
L a(JDrl u = f
121 s '"
on AN,
where a rl E C for all a. By a fundamental solution of (E), we understand a
distribution u E !?J'(IRN, C) which solves (E) in the case where 1 = b.
If u,.. is a special fundamental solution of (E), then all fundamental solutions
of (E) are obtained through
u = u,.. + v,
where v e f2'(R N , C) is an arbitrary solution of (E) with f = O.
(69a) The existence theorenz 01 Elzrenpreis (1954) and M algrange (1955). For
each equation (E), there exists a fundamental solution.
(69b) The existence theorem for the inhomogeneous problem. Let 1 E
q,'(R N , C) be given, where supp 1 is bounded (e.g., 1 is a regular distribution
corresponding to the function j E L 1.loc(A N , C) and the support of this function
is bounded). Then, equation (E) has a solution u E '(RN, C) given by
U = UF. f,
where UF is an arbitrary fundamental solution of (E).
Proof. By (68),
L atJDrl(u F . f) = L (a(JDrl uF ). 1 = lJ. f = f
(J (J
o

Distributions and Linear Partial Differential Equations
1053
(69c) Example I. The equation
U(II) = {) on R,
n = 1, 2, . . . ,
has the solution
x ft - 1
u(x) = (I' - I)!
o if x < 0,
if x > 0,
in !?}'(R, C). This is to be understood in the sense of regular distributions.
Proof. For all tp e (IR, C), set
U(q» = fR u(x)q>(x)dx.
Integration by pans yields
U(q>(II)) = t (:-:)! q>(lIt(x)dx = (-l)"lp(O).
That means U(ft)(tp) = ( - 1 r U(tpt ll ) = tp(O) = cS(lp), i.e., U(II) = b. 0
(69d) E.;-=ample 2. Table 2 shows fundamental solutions for the Laplace
equation and the heat equation (regular distributions), and for the wave
equation (singular distribution).
(70) The generalized initial value problem for the WDL'e eqrlDtion. We consider
the classical initial value problem
(C)
def A r
Du = UtI - uU = J,
x e R 3 , t > 0,
U(X, 0) = uo(x),
U,(x,O) = U I (x, 0)
Table 2
Differential equation
- Au =  on R 3
Fundamental solution
(x E (R3)
I
u(x) = I I
41l x
(regular distribution)
-IAP;41
e
Uti - 4u =- lJ on 01.
o if t s 0,
(regular distribution)
u() = 4 1 r ! ( r lp(x,')dO ) dt
n Jo t JI-'
for all tp e (R4, C)
-..... -..
8(llt)'2
if I > 0,
U r - Au = 6 on R 4
U(x, I) =

1054
Appendix
together with the so-called generalized initial value problem
(C*) DU = F + U o <8> 6' + U I (8) cS, supp U s;; ,
where  = {(x, t) e fR4: t > O}.
Problem (C.) is motivated by the following result. Let
Ie C() "0 e CI(R3 Ul E C(R3)
be given, and let U E C:Z(int A:) r. C 1 () be a solution of (C). Set U(X, t) = 0
and f(x, t) = 0 if (x, t) , .
Then the corresponding regular distributions in g)'(Ir, C) satisfy (C*).
Proof For all tp e (, C), integration by parts yields
f fPDudxdt = f fPDudxdr = - f fP(x,O)u,(x,O)dx
JR. JR JR)
+ f fP,(x,O)u(x,O)dx + f uDfPdxdt.
JA J JA
Note that cos (II, t> = - 1 and cos(n, x) = 0, where n denotes the outer unit
normal to aA: = R 3 . From (C) it follows that
f uDfPdxdt = f ffPdx - f q>,(x,O)uo(x)dx
JR. JR. JR J
+ f q>(x. O)Ul (x) dx.
JR'
This is (C*). In this connection, note that
(DU)(q» = U(Dq» = f uOq>dxdt,
JR.
F(q» = f fq>dxdt.
JR.
(VI (g)b)() = VI(b((Xt») = U1(lp(x,0»
= f ua(x)tp(x,O)dx,
JR J
(U o (g) cS')(tp) = Vo(b'((x, t») = V o ( - ,(Xt 0»
= - f uo(x)q>,(x,O)dx. 0
JR J
(70a) The Poisson formula. Our point of departure is the classical Poisson
formula:
( ) OCt) {, f(y,t -Ix - yl) d
II x, t = - y
4n b'-.¥I:5:, Ix - yl
(P) 8(t) {, 1 0 ( 8(1) {, )
+ 4 - ul(}')dO, + 4 - -;- - Uo(}') dO,. ,
nt lJ-.xI=' n lit t b'-xl-,

Distributions and Sobolev Spaces
1055
where 0(1) = 1 if 1 > 0 and 0(1) = 0 if 1 < O. Then:
(i) If U o E C 3 (R3)t U 1 E C 2 (R 3 ), and I E C2(A), then u = u(x, t) in (P) is a
classical solution of the initial value problem (C) above.
(ii) If U 09 U 1 E LI.,oc(R J ), and IE L1.loc(R 4 ) with supp I c A. then the right-
hand side of (P) represents a distribution U E '(R4, C)9 which is the
unique solution of the generalized initial value problem (C*).
In (i), the initial condition is to be understood in the sense of u(x, r) ..... uo(x)
and u,(x, I) -+ U 1 (x) as 1 -+ + 0 for all x E R J .
Distributions and Sobolev Spaces
(7Ia) The space W,"'(G). Letm = 1,2,...and 1 < p < 00. In termsofdistri-
butions, the Sobolev space Wp"'(G) can be characterized by
Wp"'(G) = {u E Lp(G): D(J U E Lp(G) for all : I{XI  m}9
where V denotes the regular distribution corresponding to the function U 9 i.e' 9
U(q» = fG u(x)q>(x)dx
for all cp E (G).
Moreover, DrJ V E Lp(G) means that D:J V is a regular distribution correspond-
ing to an Lp(G)-function. If U E Wp"'(G), then, for all 2 with II < tn, the distri-
butional derivative D rJ V corresponds to the generalized derivative D rJ u in the
sense of Section 21.1, i.e.,
(D2 U)(q» = fG (Dtlu)q> dx
for all cP E !:t( G).
(7tb) Negal;venormsandlhespace-'"(G).Letm= 1,2,...,1 <p,q< 00,
p-I + q-l = I. For V E '?'(G) we define the so-called negative norm
I U(cp)1
"UII_",., = sup .
G'eY(G) IIcplI",.p
By definition 9
 -"'(G) = {U E q'(G): II VII_",., < oo}.
(7Ic) The dual space Wp"'(G).. Choose m 9 P9 q as in (7Ib). We have
Wp"'(G)* = -"'(G)
in the following sense. Let U E Wp"'(G)., i.e., U is a continuous linear functional
on Wp"'(G) with norm IIVII. Since 9(G) c Wp"'(G),
IV(cp)1 < IIUllllcpll",.p
for all cp E P(G).
This implies U E -"'(G).

1056
Appendix
Conversely, let U e -III(G). Then,
I U()I  11 UII-III.qlltpll...p for all q> e (G).
Since (G) is dense in Wplll(G), U can be uniquely extended to a linear con-
tinuous functional on W,-(G) with
II U II = II U 11-",.,.
(71d) Representation theorem. Choose In, p, q as in (71 b). Let U(I e Lq(G) for
all a: lal s m. Let Va be the regular distribution corresponding to "a' We set
(R) U = L (-I)lalD<lU a .
13t S m
Then U E -III(G). Conversely, each U e -III(G) can be represented in the
form (R).
(7Ie) We have
...  W 2 2 (G)  wi (G) 5 L 2 (G) c W 2 - J (G) 5 W 2 - 2 (G) 5 ....
This motivates the designation "W- m ."
Distributions and Locally Convex Spaces
In (64) we defined distributions without using a topology on the space CO(G).
We only used the notion of an appropriate convergence. Now our goal is to
introduce a topology on C6(G) in such a way that distributions are linear
continuous functionals on CO(G). To this end, we need locally convex spaces
and the strict inductive limit ofF-spaces. In fact, the development of the theory
of locally convex spaces around 1950 was mainly stimulated by applications
to distributions.
The definition of locally convex spaces can be found in the Appendix to
Part I.
(72) Frechet spaces. A locally convex space is called a Frechet space
(F-space) iff it is a complete metric space, i.e., the topology is given by a
complete metric.
(72a) Standard example I. Each B-space is also an F -space.
(72b) Standard example 2-the space COC( G) . Let G be a nonempty open set
in [Ii.'l. Then CX>( G ) is an F-space. The system of seminorms is given by
p,,(tp) = L sup Il)OI«p(x)l,
pl' xc l:
for k = 0, 1, . . . . and the metric is given by
 p,,(cp - t/J)
d(q>. t/1) = tO 2 k (1 + Pt(q> _ t/1H '
for all tp, t/I E ( G ).

Distributions and Locally Convex Spaces
1057
Let Y be a real B-space. Then the linear mapping
U: COO(G) -. Y
is contillllOUS iff there exists a seminonn Pic and a constant C such that
II U(tp)U < Cp,,(cp)
for all cp e COO( G ).
(73) Strict inductive limit of F-spaces. Let X be a linear space over I< = R,
C \\'ith
oX)
X = U XII
"=1
and
X c X c...
1- 2- ,
where all the spaces X" are F-spaccs over I( and
XII is a closed linear subspace of X.. + I for all n.
Then there exists a strongest locally convex topology T on X such that all the
em beddings
XII C X,
n = I, 2, . . . ,
are continuous. We write
X = lim ind X"
II .. «-
in the case where X is equipped with the topology f. Then X is called the strict
inductive limit of (X,,) and the following hold:
(i) X is complete.
(ii) X. is a linear closed subspace of X for all n.
(iii) Let Y be a locally convex space over 1<. Then the linear mapping
U: X -. Y
is continuous iff the restrictions
U:X.-+Y
are continuous for all n.
(73a) Explicit construction of t. Let S  x. Then the set S is called circled
iff, for )" e 1(,
.'t e S
and
1).1 < I
implies
Ax e S.
Moreover, S is called absorbing iff
U tS = X.
r:> 0
Let t be the system of all convex, circled, absorbing sets S in X with the
additional property
s " X. is open in XII for all n.
Then a set J\1 in X is open with respect to the inductive topology t iff, for each

1058
Appendix
X E M, there exists a set S E 1: such that
x + S C M.
(73b) Strictly inductive topology on Co(G). Let G be a nonempty open set
in (RN. We choose a sequence (G,,) of nonempty open sets G" in R N such that
:xJ
G = U G"
,,=1
and
- -
G C G c"'c G
1- 2- ·
We set X = CO=)(G) and
x" = {tp E X: sUpptp c G,,}.
Then X" is a closed linear subspace of the F-space CX)(G..), i.e., X" is an F-space.
Thus, we have the same situation as in (73). We now equip C\(G) with the
corresponding inductive topology t, i.e., we set
Co(G) = lim ind C'XJ(G,,}.
lI"'tXJ
Let Y be a real B-space and let
U: C(G) -. Y
be a linear mapping. Then the following three conditions are mutually
equivalent:
(i) U is continuous.
(ii) U is a distribution in the sense of (64).
(iii) For each n E N, there is a constant C and a number k = 0, I, ... such that
II U(cp)II  C L sup ID<ltp(x)1
II  It x E G"
for all cp E CCXJ( G,,).
An analogous result holds for distributions U: (G, C) --+ Y with values in
a complex B-space Y. In this connection, one has only to replace the real
C.(G)-functions by complex Co(G)-functions, i.e., replace (G, R) by !I}(G, C).
Fourier Transform and Sobolev Spaces
(74a) The space .C/. We set
PI1.m(u) = sup (lxlA: + I) L IDu(x)l,
oX E R N Iell  1ft
where k, m = 0, I, ... and N = I, 2, .... The space .(RN) consists of all the
C)-functions u: IR N -+ C with
Pi.",(U) < 00 for all k, m,
i.e., the functions U E Y(IR N ) and their derivatives are rapidly going to zero as
Ix I -. 00. In particular,
(RN, C) c ,(RN).
The function u(x) = e- 1x11 belongs to ,Y'(R N ), but not to (RN, C).

Fourier Transform and SoboJc\' Spaces
1059
.9'(R''i) is an F-space equipped with the seminonns {Pt,,,,}. We have
U II ..... U on 9'(IR N ) as n --. 00
iff P".m(u.. - u) -. 0 as n --. 00 for all k, In.
(74b) The Fourier transforna. Let u e 9'(R"'). The classical Fourier trans-
form Ii = Fu is given by
A ( ) 1 r -,(,Ix> ( ) d
u y = (21tt"/2 JRIr e u x x.
This transformation, which represents one of the most important tools in
analysis, has the following three fundamental properties:
(i) l,averse transfornlation. The operator
F: 9'(Ra) -+ Y(IR N )
is a linear homeomorphism. The inverse transformation II = P-l rl is
given by
u(x) = _1_ r e'(,Ix>a(y)dy.
(2nf 1 2 JRN
(ii) Differelltiation passes to multiplication. From the last formula we obtain
the key relation:
D2 U ( X ) = I r ei<1IJt>illll y u (y) d y
. (21t)N / 2 J Rill ·
More precisely, for all multi-indices « = (1" .., ,..) and all u E .9(R"'),
we obtain
F(J)2u) = i bl }1 C1 Fu.
Conversely,
J)2(Fu) = ( - i F(xGlu).
where }' = ("1" . . , 'IN) and y41 = 'Ii' '12 2 . . . ,,'f.
(iii) Invariance of the scalar product. We have the so-called Parse\'al identity
(FuIFv) = (ulv)
for all u, v e 9'«(RH),
where (ulv) = JRlv u vdx.
(74c) Extension of the f"our;er transform. Since 9'(R N ) is dense in L(Ra'l),
the Fourier transform F: .«(RN) --. V(R"') can be uniquely extended to a
ulJitar}1 operator
F: L(R') ..... L(R"')
such that (FuIFv) = (ulv) for all u, v E L(IRN).
(74<1) The Sobolev space W 2 '"«(RN), m = I, 2, ..., consists of exactly all the

1060
Appendix
functions 14 E Lz(RN) with
II u":. 2 dcr (fA" (1 + I Yl2 )"'1 u(Y)1 2 dy Y/2 < 00,
where'u = f'u. Furthermore, /I'U:.2 is an equivalent norm on W 2 m (R N ).
If 14 E W 2 '"(R N ), then Dtlu E L 2 (R N ),
F(DtJ 14) = i 'lll yll Fu,
and F(DtJu) E L(RN) for all a: lal  m.
(74e) The dual space g"(R N ). This space consists of all continuous linear
functionals
T: .5I'(R N ) -+ C.
Each TE .'(RN) is called a tempered distribution. The Fourier transform
F: Y'(RN) --+ Y'(RN)
is defined by
(FT)(cp) = T(Fcp)
for all cp E (IRN).
A linear map T: .(RN) --+ C is a tempered distribution iff there exists a
seminorm PIc.m and a constant c such that
I T(cp)1 < CPIc,m(CP) for all cP E .(RN).
(74f) Examples. The following functions u: n N --+ C belong to Y'(R N ):
(i) 14 E L(AN), I  p  00;
(ii) 14 = polynomial.
The corresponding distributions Te .9'(R N ) are given by
T(cp) = f ucpdx for all cp E 9'(IR N ).
JRN
If there exists a measurable function 14: R N --+ C with
f ucpdx = f uFcpdx for all cp E 9'(R N ),
J R.'i J AN
then the Fourier transform FT of T can be identified with u in the sense of
FT(cp) = f ucp dx
JRN
for all cP E .</(R N ),
We briefly write Fu = u.
(74g) Further examples. We have
Y"(R N ) £; '(RNt C).
The following distributions from '(RN, C) also belong to .'(RN):

l.he Sobolev Sraces ",. for Real m
1061
(i) the delta distribution b and all its derivatives D<lb;
(ii) D 2 u for u E Lp(R'\'), 1 < p < w, and arbitrary ex.
This is to be understood in the sense of
b( cp) = cp(O), (D<I b) (cp) = ( - 1 )1:zlb(D<I cp),
(D 2 u)(cp) = ( - 1)100Iu(DOIcp) = (- 1)121 r uDOIcp dx.
JA
for all cp E .'l' (R.\'). Moreover,
f'b = (2n) - ,\','2.
This follows from
Fc5(cp) = c5(Fcp) = (Fcp)(O) = r (2nr Nl2 cpdx.
JAN
for all cp E /I'( R N ).
The Sobolev Spaces Wpm for Real m
We summarize the basic definitions for real and complex Sobolev spaces WI''''
of arbitrary real order m. For noninteger m, the spaces Wp'" are also called
Sobolev-Slobodeckii spaces. Below we will consider the connection between
the spaces W 2 '" and the Fourier transform.
Let 1 < p < OC I withp-J + q-J = l,i.e.,p = 1 impliesq = oc\. Furthermore,
let G be a nonempty open set in R N , N > 1. Finally, let I( = R, C. We set
f( ) - f. fu(x) - u(y)fP d d
u - --- x '
G x Ci I x - ylN+ Pli . ,
II U 1/ "'. P = ( r r I D 2 u I P d X ) II P .
lell S '" J G
IIull i . P = ( IIUII.p + r f(D<lU) ) I!P.
1<11 S '"
where m = 0, I, . . . , and k = m + J,l, 0 < Jl < I.
( 74 h) Le t m = 0, I, . .. . We se t
W;(G)t( = {u E L(G): D"u E L(G) for all ex: 'I  In}.
This space together with the norm lI'II""p becomes a B-space over IK.
(74i) Let k = nJ + 11, m = 0, I, . . . , and 0 < Ji < I. We set
H'p' ( G h = {u E J.t'" ( G)K: II u II i. P < ex;}.
This space together with the norm /1'11,. p becomes a B-space over IK.

1062
Appendix
(74j) For real m > 0, let
Wp'"(G)" = closure of !?}(G, IK) in Wp'"(G)K
and
-'"(G)K = Wp'"(G).
As usual, the star denotes the dual space.
If IK = IR, then we set Wp'"(G) = Wp'"(G)A.
(74k) Let -00 < I  m < 00. Then the embeddings
(RN, C) c Y(R N ) £; W 2 '"(R N )c £; Wl(R N )c
C 9"(R N ) C '(RN, C)
are continuous provided the dual spaces 9"(R N ) and ,q}'(R N , C) are equipped
with the weak. topology (cr. A.(41)).
(741) Let m > I + N /2 with I = 0, 1, . .. and suppose that G is a bounded
region in R N with iJG E Co. .. Then the embedding
W 2 '"(G)1( £ C'( G )K
is continuous. This also holds true if either G = R N or G is an open half-space
in R N . Here, the space C'(G)I( consists of all continuous functions u: G -+ IK
with
II u II, def L sue I DClU(x)1 < 00.
ICiI  I J( E G
In this connection, we assume that all the derivatives DCl U : G ..... It( are con-
tinuous up to the order I and that these derivatives can be continuously
extended to the closure G.
The Sobolev Spaces W 2 m for Real m and the Fourier Transform
Let N > I, and let m be a real number. We set
H'" = W 2 '"(R N ),
He = W2'(R N )c.
(74m) Let
(ul v):. 2 = [ u(y) O(y)( 1 + lyI2)," dy
JRN
and let lIull:.2 = (UIU):.12, where u denotes the Fourier transform of u, i.e., .
lIull:.2 = (fA" lul 2 (1 + IYI2)," dy ) 1/2.
Then
He = {ue9"(R N ): lIull:.2 < oo}.

Construction or Measures and the Main Theorem of Measu Theory
1063
More precisely, the space He consists of all the distributions u in !/' whose
Fourier transform al is a function with lIull:.2 < 00.
(74n) The space He together with the scalar product (1IIv):.2 becomes a
complex H-space. The set (RN, C) is dense in He.
(740) The norm 11.11:.2 is an equivalent norm on W 2 111 «(R''')c.
(74p) For all m  0.
H-'" = (H'")*, He'" = (He)..
(74q) Interpolation. Let r, S E III with s < r and let
m = (1 - O)r + Os,
Then the embeddings
o < 0 < I.
He c He c H
are continuous, and for all II e He and all I: > 0, we have
111111:. 2  Ilull  -'II ull
and
II u II:. 2  t II u II  2 + t 1 -11' II U II 2'
In the sense of(112) below, we obtain
[H c , HC]S.2 = He,
where the corresponding norms are equivalent.
Construction of Measures and the
Main Theorem of Measure Theory
The concept of measure is one of the most important tools of the mathematics
of the twentieth century. For example, measures play an important role
in the theory of probability. spectral theory, and representation theory of
topological groups (together with applications in elementary particle physics).
One distinguishes between
(i) measures on arbitrary set and
(ii) measures on topological spaces.
Primarily, the notion of measure has nothing to do with topology. Therefore,
we use here an approach to measure theory which works on arbitrary sets
without any topology. In particular, such an approach is important \\'ith
respect to the general theory of probability. We follow the line:
premeasure  measure  integral.
The main theorem of measure theory, (7Sf) below, contains a construction for

1064
Appendix
the unique extension of a premeasure to a measure. If we apply this construc-
tion to the volume of cuboids in R N , then we obtain the classical Lebesgue
measure on R H . Similarly, one obtains measures on curves (arc length) or on
surfaces (surface area).
The integral J f dJJ with respect to a measure JJ is defined completely anal-
ogously to the Lebesgue integral J f dx in (14).
Measures on topological spaces will be considered in (83fT). In this connec-
tion, the representation theorem of Riesz- Markov and the compactness
theorem of Prohorov are especially important. In particular, the Prohorov
theorem is needed for the investigation of the Navier-Stokes equations by
means of stochastic procees.
(75a) Set algebras and u-algebras. Let S be a set. By definition, a set algebra
of S is a nonempty system " of subsets of S which is closed with respect to
the usual finite set operations, i.e., if the sets At B, A I' . . . t A", belong to .w,
then the sets
S - B,
A - B,
'"
U A",
,,=1
'"
n A",
,,-1
also belong to .rN. In particular, S and the empty set belong to /N.
By definition, a a-algebra .rJ is a set algebra which has the additional
property that the sets
\X)
CIJ
n A"
,,=1
U A",
,,=1
belong to d provided all the sets A" belong to d.
Let .r4 be a set algebra of S. Then a(,-) denotes the intersection of all the
a-algebras of S which contain ..rd. This intersection is nonempty and a(.f;{) is
the smallest a-algebra of S with a(r¥) ::J .91.
Example. Let X be a topological space. By definition, the Borel field fM(X)
is the smallest a-algebra of X which contains all the open sets in X.
(75b) Measure. Let Ltt be a a-algebra. By definition, a measure on d is a
function
JJ: .W ..... [0, 00]
with the following two properties:
(i) Additivity. Let A and B be two arbitrary disjoint sets from .91. Then
JJ(A u B) = JJ(A) + JJ(B).
(ii) a-Additi(,ity. Let {A,,} be an arbitrary countable family of pairwise disjoint
sets A" from .r:I. Then
Il CQ An) = n Il(A n ).

Construction of Measures and the Main Theorem of Measure Theory
1065
The number p(A) is called the measure of the set A. In terms of physics,
measures may be interpreted as mass or charge. In the theory of probability,
measures correspond to probability.
From the a-additivity of a measure Il we obtain the following two continuity
properties:
lim ( 0 AI ) = P ( U Ai ) '
,,"'-x- 1:1 t:=1
lim ( {1 Ai ) = /J ( .f1 Ai ) .
"...«- ,=1 .=1
Here. it is assumed that all At belong to ..t(.
(7Sc) Premeasure. The notion of a premeasure is weaker than the notion of
a measure. Let ..tI be a set algebra. By definition. a premeasure is a function
p: . -+ [0,00]
with the following two properties:
(i) Additivity. Let A and B be two arbitrary disjoint sets from d. Then
p(A u B) = p(A) + p(B).
(ii) Weak O'-additivity. Let {An} be an arbitrary countable family of pairwise
disjoint sets from . so that the union U:-l A" also belongs to d. Then
JlCQ AR) = Rt p(A n ).
Special properties of measr4res. Let d be a a-algebra of S. A measure JJ on
r!/ is called finite iff peS) < 00.
A measure on .91 (or a prcmcasure on the set algebra .91) is called a-finite
iff there exists a decomposition of the form
oc.
S = U SII
11:1
with Sit e d and p(S,.) < 00 for aU n.
If p(A) = 0, then A is called a zero set. In particular, p(0) = O.
(7Sd) Standard example and motivation. Intuitively, the prototype of a mea-
sure is given by a point Xo in AN with mass m > O. To be precise, let ..vi be the
system of all sets in lR'v and define
{ m
Po(A) =
o for .o f. A.
Then Po is a measure on the O'-algebra JI'I. PhysicaUy, Po(A) is the mass of the
set A (Fig. 6 for N = I).
for Xo e A,

1066
Appendix
A
,\ m
./ &
Xo
Figure 6
In order to obtain a continuous mass distribution on fR"', we choose a
continuous function p: IR''I -. iii... and define
Il(A)::: f.. p dx,
where the integral is to be understood in the classical sense. Then we regard
Il(A) as the mass of the set A, and we regard p(x) as the mass density at the
point x. The point is that the classical integral fA p dx only exists for sufficiently
regular sets A. Thus, we do not obtain a measure but merely a premeasure.
However, by the following main theorem of measure theory (15f), this pre-
measure can be uniquely extended to a measure which is called the Stieltjes
measure.
In physics, the concept of measure has the advantage that one can handle
discrete and continuous mass distributions in a uniform way. In the physical
literature one writes formally
llo(A) = f.. m<5(x - xo)dx,
where the "delta function" "x t-+ b(X - xo)" is called the mass density of a
discrete mass m = 1 at the point x = Xo.
(15e) ConJplet;on of a measure. A measure p on d is called complete iff every
subset of a zero set is again a zero set, i.e., for aU A E d,
peA) = 0 and B C A imply B e. and pCB) = O.
Let It be a measure on the a-algebra d Then there exists a smallest a-algebra
. such that p can be extended to a complete measure on .91. This extension
is unique. Here, ..t1I is called the completion of d with respect to Jl.
Explicitly, , consists of all the sets
AuZ,
Aed,
\vhere Z is an arbitrary subset of a zero set. Then the extension of p to $/ is
given by peA u Z) = peA).
(75f) The nwin theorem of measure theory. Let It be a a-finite premeasure
on a set algebra .91. Then JJ can be uniquely extended to a measure on q(Jaf).
Moreover, this measure is a-finite.

Construction of Measures and the Main Theorem of Measure Theory
1067
A
A 2
".igure 7
(75g) Idea of proof. The construction of the measure is based on the
following steps.
Step I: Outer measure Jl..
Let Lrd be a set algebra of the set S and let A c S. We choose an arbitrary
and at most countable family {All} of sets with
A c U A"
and
A" E .cI
for all n.
"
Let
a = L Jl(A,,).
"
By definition.
Jl.(A) = infa,
i.e., the outer measure Jl*(A) of A is equal to the infimum of all possible
numbers a (Fig. 7).
Step 2: a-algebra J.l.(c).
Let A £ S. By definition, the set A belongs to Jl*(tI) iff
Jl.(M) = JI*(M (\ A) + Jl.(M - A) for all subsets hI of S.
Step 3: Measure Jl.
We set
J.l(A) dcr Jl.(A)
for all A E J.l*(,c.{).
Then we have a(d) £ J.l.(d) and the restriction of Jl to 0'(.") is the desired
measure.
Step 4: Completion of the measure J.l on a(.Qf).
One can show that this unique completion in the sense of (75e) coincides
with Jl on Jl.(.Q/).
(75h) Standard example 1. Lebesgue measure on R'IV. N > I. Let -  <
a i < b i  00 and x = (I'.'" N)' Exactly all the sets
J = {x E R N : a; < i < b i for all i}

1068
Appendix
( - - --

-- ----.
N == I
N=2
J."'igure 8
------
M -----
-----.,
I
I
I
Figure 9
are called half-open intervals in (RN (Fig. 8). We set
N
Jl(J} = volume of J = n (b i - a).
i I
In order to extend Ji to a measure, let .9/ be the system of all the sets of the
form
M = U J",
"
where {I n } is an arbitrary finite family of pairwise disjoint half-open intervals
J II in R N (Fig. 9). We set
Jl(M) = L JJ(J,,)
"
and J.l(0) = O. Then J.l is a a-finite premeasure on the set algebra .".
We now use the main theorem of measure theory (75f), (75g). The extension
of Jl to the a-algebra J.l.(w) is called the Lebesgue measure on R N . 
This measure is a-finite and complete. Moreover, the a-algebra a(.s;{) coin-
cides with the Borel field (RN). Note that a(d) c JJ.(.).
(75i) Standard example 2. Stieltjes measure on R. Let M: R -+ R be a mono-
tone increasing function which is continuous from the left (Fig. 10). Let
J = [a, b[ be an half-open interval. We set
JJ(J) = M(b) - M(a).
In the case where a = -00 or b = 00 this is to be understood in the sense of
a corresponding limit.
We now use the same construction as in (75h). Then the corresponding
extension of JJ to a measure on Jl.(d) is called the Stieltjes measure. This

Measurable Functions on Measure Spaces
1069
M
./
II
t-
b
.
Figure 10
measure allows the following physical interpretation:
(A) = mass of the set A.
In particular. ajump of the function M at the point a corresponds to a discrete
point mass at a, i.e..
I( {a}) = lim M(b) - M(a)
b-a+O
(cf. Fig. 10). In the special case where M = 1 we obtain the Lebesgue measure.
(75j) Measure space. By definition. a measure space (S, Lr#.I) is a triple
consisting of a set S. a a-algebra cf of S, and a a-finite measure IJ on r:Y.
A measure space is called complete (resp. finite) iff the measure IJ is complete
(resp. finite. i.e.. IJ(S) < oc).
The sets A in .cf are called measurable.
In the following we shall see that many results for the classical Lebesgue
measure can be generalized to measure spaces. This is very important for many
branches of modern mathematics and mathematical physics.
Measurable Functions on Measure Spaces
Let (S, ,ct, IJ) and (T,, ,,) be measure spaces and let Y be a B-space. Note that
there exist two different notions of measurable functions in the literature which
will be introduced in (76a) and (76b) below. The relation between these two
notions will be described in (76c) and (76<1). Let M c s.
(76a) Measurable functions. Completely analogously to (7), a function
f: M --+ Y
is called measurable iff M is measurable, i.e., M E .eI, and there exists a
sequence (f,,) of step functions f,.: M --+ Y with
f(x) = lim f,.(x)
for almost all x E AI.
"-J

1070
Appendix
(76b) p-Measurable functions. The function
f: M ..... T
is called p-measurable with respect to d and .'M iff
BE implies f-I(B)E&W.
This notion is important for the theory of probability.
(76c) Theoretn. Let the B-space Y be separable (e.g. Y = IR) and let the
measure space (S, JII, JJ) be complete.
We consider the function
f: M ..... Y,
M E ..r4.
Then the following three conditions are mutually equivalent:
(i) f is measurable.
(ii) f is p-measurable with respect to..fl1I and the Borel field EM( Y), i.e., B E (Y)
implies f -I (B) E ...
(iii) The preimages of open sets are measurable.
(76d) Modified theorem. Suppose that the B-space Y is not necessarily
separable. Then we need the following assumption:
(A) There exists a zero set Z such that f(M - Z) is separable, i.e., the set
.f(M - Z) contains an at most countable dense subset.
Then theorem (76c) must be replaced by the following equivalences:
(i)  (ii) + (A)  (iii) + (A).
(77) Theorem of Egorov. Let (S, d, JJ) be a complete measure space and let
Y be a B-space. Suppose that the sequence (f,.) of measurable functions
fn: M ..... Y
converges almost everywhere on M to the function f: M ..... Y, where JJ(M) <
00. Then:
(i) j' is measurable.
(ii) (fll) is uniformly convergent up to sets of small measure, i.e., for each tJ > 0,
there exists a subset M6 of M such that JJ(M 6 ) < lJ and
f,.  f on M - M6 as n..... 00.
Integrals with Respect to General Measures
Let (S, ..r;;/, Jl) be a measure space and let Y be a B-space. We consider functions
f: M c S  y

Integrals with Respect to General Measures
1071
with M E .r.f. Completely analogously to (14), the integral J,.,f dJl is defined
by the formula
r f dJJ = lim f fn dJj,
J,., ,,-oc M
where (f..) is a sequence of step functions fIt: M --+ Y.
In the case where M = 0 we set JAIl dp = O.
(78a) Properties of the integral. Completely analogously to the classical
Lebesgue integral, the following theorems are valid for the integral J", f dll:
(i) Majorant criterion (17).
(ii) Norm criterion (18).
(iii) Majorized convergence (19).
(iv) Generalized majorized convergence, monotone convergence, and the
lemma of Fatou (19a)-(19c).
(v) Absolute continuity of the integral (20).
(vi) Continuity and differentiability of parameter integrals (25a), (25b).
The theorem of Fubini- Tonelli on iterated integration and the theorem of
Radon - Nikodym on absolutely continuous measures can be found in (81)
and (82), respectively.
(78b) u-Addit;l'ity. Suppose that M =. U" M", i.e., the set M is the union of
an at most countable family of pairwise disjoint measurable sets M". Then
f f dJJ = r. r f dJJ.
AI " J",,,
Here, the existence of the left-hand integral implies the existence of all the
right-hand integrals and the convergence of the corresponding series.
(78c) Cont'ergence M,;th respect to the domain. Let M 1 c M 2 c: ... be a
sequence of measurable sets with
M" -+ M
as n -+ 00,
i.e., M = U" M". Then
f f dJJ = lim r f dp.
AI " -. 00 J AI"
Here. the existence of the left-hand integral implies the existence of all the
right-hand integrals and the existence of the limit.
(79) The Lebesgue space Lp(M -+ Y, Il), t $ p < 00. We set
IIfll p = (fM IIf(x)IIPdJJ YIP.
Let Lp(M --+ Y,p) denote the set of all measurable functions f: M --+ Y with
II/lip < 00.

1072
Appendix
Then Lp(M --+ Y, JJ) together with the norm II-Up becomes a B-space once we
identify any two functions which differ only on a zero set in M.
In the case where Y = IR and Y = C we write briefly Lp(M, JJ) and L(M, JJ),
respectively_ J..or p = 2, these two spaces are H-spaces with the scalar product
(fIg) = 1, Jg dJl.
More generally, if Y is an H-space, then L 2 (M --+ Y, JJ) is also an H-space with
the scalar product
(fig) = f", (f(x)lg(x)) dJl.
(79a) Density. The set of step functions f: M  Y is dense in Lp(M  Y, JJ),
I  p < oc.
(79b) The Holder inequality. Let 1 < p, q < 00, p-l + q-l = I and let Ie
Lp(M --+ Y, JJ), g E Lq{M --+ Y, JJ). Then
f", IIJ(x)lIlIg(x)1I dJl  IIJllpllgll".
(79c) Convergerace of subsequences. Suppose that
f,. --+ f in Lp(M -+ Y, JJ)
as n -+ 00
and I < p < 00. Then there exists a subsequence with
/,..(x) --+ f(x)
as n --+ 00 for almost all x eM.
(79d) Convergence in measure. Let f: M --+ Y be a measurable function and
let (f,.) be a sequence of measurable functions /,.: M --+  By definition, (/,.)
converges to f in measure iff
lim JJ(IIJ:. - III > c) = 0
for all c > o.
,.-)
To be precise, this means JJ(M,.) -+ 0 as n -+ 00 for all £ > 0, where M,. =
{x E M: II/"(x) - f(x)1I > £}.
In the theory of probability one uses synonymously:
convergence almost everywhere = convergence almost certainly,
convergence in measure = stochastic convergence.
(7ge) Interrelations between different notions of convergence. Let (S, d, JJ) be
a measure space and let Y be a B-space. We consider an arbitrary sequence
(I,.) of measurable functions
/,.: M  Y.
Then all the statements of Figure 11 are valid. For example, convergence
almost everywhere implies convergence in measure if JJ(M) < 00, etc.

Product Measures
1073
uniform
convergence on M
l
pointwise
convergence on M
t
convergence in the
space Lp(M  Y.).
ISp<oc
(p-mean convergence)
convergence
almost
everywhere on M
(almost certainly)
pI"') < ex: rIM) < ex:
convergence pC A') < «..
in measure on M
( Rtesz )
(stochastic
convergence)
p ,,-ompJctc, pC AI) < <r)
uniform convergence
..
up to small sets
(E80'0\')
existence of a
. subsequence which
converges almost
everywhere
Figure 11
In particular. we may choose S = (R.'1, P = Lebesgue measure, and M =
bounded open set in R.... Then p is complete and p(M) < 00.
Product Measures
(80) Defillition. Let (S,, Il) and (1: &f, \.) be measure spaces. We construct a
new measure space (product space)
(S x T:.. x &f,Jl X \t)
in the follo\\'ing way:
(i) d x  denotes the smallest a-algebra of S x T \\'hich contains all the
product sets
AxB
(ii) For all A e .91, B e EM, we set
(Il x \t)(A x B) = Il(A)\'(B).
with A e SIll, B e .
Then there exists a unique extension of Il x v to the a-algebra .91 x 91. This
measure is called the product measure Il X".
(80a) Explicit construction of ti,e product measure. Let C E.rtI x ffI. We
choose arbitrary sets A. e gf and B" E  with
tTJ
C  U An X B.
,,-'1

1074
Appendix
and set
CXJ
a = L (AII)v(BII).
11=1
Then
(/J x v)(C) = infa,
i.e., (/J x v)(C) is equal to the infimum of all the possible numbers a.
(80b) Example. Let S = T = IR and /J = v = Lebesgue measure on R. Then
d x  contains the Borel field (R2) and the product measure  x v is equal
to the Lebesgue measure on" x . The completion of this product measure
yields the Lebesgue measure on R 2 .
An analogous result holds for RII x R"'.
(8Oc) Product n,easure for arbitrarily many factors. The following construc-
tion due to Kolmogorov is fundamental for the theory of stochastic processes.
By definition, a probability space is a measure space (E, .91, p) with (E) = 1.
We are given a system of probability spaces
(Ej'L,/Jj)' j E J,
where J is an arbitrary finite or infinite nonempty index set. We want to
construct a probability space (E, .,) for the product set
E = n EJ.
j
(i) We construct .r4 = nJ .. A set C in E is called a cylindrical set iff
C = n Aj
j
and Aj ;f.: Ej holds for at most finitely many j. Let nJ  denote the smallest
a-algebra of E which contains all the cylindrical sets of E.
(ii) We construct  = njpj. For all cylindrical sets C, we define
Jl(C) = n Jl(AJ).
j
By the main theorem of measure theory (75f), the premeasure /J can be
uniquely extended to a measure  on ..
(81) The theorem of Fubini- Tonelli. Let (S,d,) and (J:, v) be measure
spaces and let the function
f: S x T -+ R
be p-measurable with respect to sI x 91 and the Borel field (R), i.e., the
prcimages of open sets are measurable with respect to d x . Then the
following two conditions are equivalent:
(i) JS)( T f d(  x v) exists.
(ii) Js (J T I f(s, 1)1 dv(t» dJl(S) exists.

Absolute Continuity of Measures
1075
If (i) holds, then
ISTf d(p x v) = Is (IT f d')d P
= IT (!sf dp )d".
Absolute Continuity of Measures
Let (St ..(j. JI,) and (S. d. \') be measure spaces.
(82a) The theorem of Radon-NikodynJ. The following two conditions are
equivalent:
(i) There exists an integrable function f: S -. R such that
\'(A) = If dp
for all A e d.
(ii) The measure v is absolutely continuolls with respect to JL, i.e. t by definition,
Jl(A) = 0 implies v(A) = O.
Moreover. if (ii) holds, then f in (i) is unique as an element of the space
L 1 (S, p).
(82b) The decomposition theorem of Lebesgue. The measure \' allows a
unique decomposition
v = V ac + vsl n .
with the following properties:
(i) The measure "ac on d is absolutely continuous with respect to the given
measure p on .91, i.e., Il(A) = 0 implies "ac(A) = o.
(ii) The measure "sinl on &I is singular with respect to ", i.e., there exists a set
A E sf such that p(A) = 0 and VSiD1(S - A) == O.
Using the Radon-Nikodym theorem (82a), we may write
\'(A) = If dp + "lnl(A) for all A e .fJ/,
wherefe La(S,p) is uniquely determined by the measure v.
(82c) Special measures. A measure Jl on the O'-algebra ..(/ is called a pure
point measure iff
p(A) = L p({x})
A
for all A E ..'J/.

1076
Appendix
The measure Ii is called continuous iff
peA) = 0
for all onc-point scts A.
A set A E ..c( is called an alOIn iff
p(A) > 0
and there is no subset B  A with 0 < p(B) < Il(A). The measure is called
nonatolnic iff there arc no atoms. For example. the Lebesgue measure on R'v
is nonatomic.
(82d) Tile tlleoreIJ. of Ljapunov 01' '-''eCIOT nJeaSllres, Let (S, .tI, Il),j = I,..., n,
be a family of finite measure spaces, i.e., Jl)(S) < 00 for all j. The family
('ll t' · · t Ii,,) is called a t'ector Ineasure with values in IR" and the set
R = {{Ill (A), · · ., p,,(A»: A E d}
is called the range of the vector measure.
Then the range R is a compact convex subset of Gi" whenever each Pj is
nonatomic.
This theorem plays an important role in modern control theory (Bang-
Bang control).
Measures on Topological Spaces
Measure theory on topological spaces culminates in the Riesz- Markov
theorem (87) below. Roughly speaking, this theorem says that on compact
spaces, continuous functions and measures are in dual;,)'_ In the following let
X denote a topological space.
(83) Basic lJot;ons, Figure 12 contains interrelations bet\veen important
classes of topological spaces. The corresponding definitions may be found in
the Appendix of Part I. In particular, a topological space is called locall)'
compact iff every point has a compact neighborhood. A locally compact space
is called countable at in/i,aity iff it is the union of at most countably many
compact subsets. The prototype of such a space is the RN.
Let C(X) denote the set of continuous functions
.f: X  R
and let Co(X) denote the set of all functions f e C(X) with compact support.
\\'e set
IIflle = max If(x)1
xx
for all fe Co(X).
If X is compact, then C(X) = Co(X).

Measures on Topological Spaces
1077
compact space
with a countable basis
I
locally compact .
with a countable basis
R"
compact
locally compact
and countable
at infinity
I Polish space I
(completely metrizable
"'ith a countable basis)
I normal I ...
metrizable
t
F -space
f
B-space 
I separable B-spacc I
..eigure 12
(84) Borel sets and Baire sets. By definition, the Borel field £V(X) is the
smallest a-algebra of X which contains all open sets of X.
By definition. the Baire field o(X) is the smallest a-algebra of X \vhich
contains all the preimages of open sets with respect to all the functions
Ie C(X). Obviously,
o(X)  (X).
The sets in £fo(X) and aI(X) are called Baire sets and Borel sets, respectively.
(i) o(X) is the smallest a-algebra ..tI of X with the property that all con-
tinuous functions f: X -+ Rare p-mcasurable with respect to d and £j'(H).
(ii) Let X be a normal space. Then the Baire field ao(X) is the smallest
a-algebra of X which contains all the open sets 0 of the form
o = U C II ,
"
where {C II } is an at most countable family of closed sets C II .
Note that, by Figure 12, both compact spaces and metrizable spaces
are normal. In particular, the (R''i and B-spaces are normal.
(iii) Let X be a metrizable space. Then alo(X) = tM(X), i.e.,
Borel set = Baire set.
Note that the R'" and B-spaccs are mctrizable.

1078
Appendix
Let X and Y be topological spaces. Then a function f: X --+ Y is called a
Borel function iff the prcimages of Borel sets are again Borel sets.
(85) Borel Ineasure and Baire nleaSllre. A measure JJ on X is called a Borel
measure (resp. Bairc measure) iff it is defined on the Borel field (resp. Baire
field) of X and
Jj(C) < 00
holds for all compact sets C in X.
A Borel measure }J on X is called regular iff, for all Borel sets S, the measure
Jj has the following two important approximation properties:
Jj(S) = inf{Jl(O): S c 0,0 open},
Ji(S) = sup{Jl(C): C c S, C compact}.
Analogously. a Baire measure Ji on X is called regular iff for all Baire sets S,
Jl(S) = inf{ Jj(O): S c 0, 0 open Baire set},
JJ(S) = sup {JJ( C): ( c S, C compact Baire set}.
(i) Let X be a Polish space. Then:
Borel set = Baire set,
Borel measure = Baire measure = regular measure.
This underlines the importance of Polish spaces for measure theory. Note
that each separable B-space (e.g., the IR N ) is a Polish space.
(ii) Let X be a metrizable space. Then:
Borel set = Baire set,
Borel measure = Baire measure.
(iii) Let X be a locally compact space which is countable at infinity. Then:
Baire measure = regular and a-finite Baire measure.
Moreover, if JJ is a Baire measure, then Co(X) is dense in Lp(X,JJ) with
1 < P < 00.
(iv) Let X be compact. Then each Baire measure is regular and can be
uniquely extended to a regular Borel measure.
(85a) Standard example. The Lebesgue measure on IR N is a regular Borel
measure and a regular Baire measure. Here, Borel sets and Baire sets are the
same.
(86) By definition, a positive linear functional on Co(X) is a linear functional
L: Co(X)  IR such that
f > 0 on X
implies
L(f) > o.

Mea..urcs on Topological Spaces
1079
If X is locally compac then every positive linear functional on X is locally
bounded, i.e., for each compact set K in X, we have
I L(f)1  const IIf"c,
for all Ie Co(X) with suppl c K.
(87) TheorenJ of Riesz-- J\f arkov. Let X be a locally compact space which is
countable at infinity.
Then there exists a bijective mapping L.-+ /J between the positive linear
Cunctionals L on Co(X) and the Baire measures p on X such that
L(/} = Ixl dp
for all f e Co(X).
(87a) Tile dual space C(X, K)*. Let X be a compact space and let 0( = R,
c. Moreover, let C(X, K) denote the set of all continuous functions I: X ..... 1(.
We set
11/11 = max 1/(x)l.
f:X
Then C(X, 1<) together with the norm n.n becomes a B-space over 1<. Exactly
all the linear continuous functionals F: C(X, K) -+ (K are given by the formula
F(/) = Ix I dp
for all Ie C(X, 0<).
In this connection, we have
{ JJI - Jl2
P=
PI - P2 + i( P3 - 1'4)
where all the Pj denote Baire measures on X.
Moreover, all the I'j are uniquely determined by F.
In this connection, we use the convention
for IK = R,
for IK = C,
f f dp = L 2) f f dPJ
x J x
if I' = La.Jl'j' where the "J are complex numbers and the Pi are measures.
(88) Vague and weak. contrgence of Haire measures. Let JI o(X) denote the
set of all Baire measures on X and let C,,(X) denote the set of all bounded
continuous functions f: X -+ R. Moreover, let Pit' JJ e .,I( o(X). By definition,
vague
Pn · JJ
as n-.cc
iff
Ix I dp" - Ix I dp
as n  00 for all f e Co(X).

1080
Appendix
Similarly,
weak ·
JJ" · JJ
as n -. 00
iff
Ix I dJl" -+ Ix I d
Since Co (X) £; Cb(X),
as n --+ 00 for all IE Cb(X).
" weak"  implies " vague. .
(88a) By definition, the vague topology on ..H o(X) (resp. weak. topology) is
the coarsest topology on ..Ho(X) with the property that for all IE Co(X) (resp.
Ie Cb(X)), the mapping
J' Ixi d
is continuous on Ho(X).
Explicitly, a set S in  IIo(X) is open with respect to the vague topology (resp.
weak. topology) iff, for each JJo E S and for each E; > 0, there exists a finite
number of functions /; E Co(X) (resp. /; E Cb(X)) such that all the measures
JJ E . ,It o(X) with
sp Ix J; dJl - Ix J; dJlo < E
belong to s.
(88b) Vague compactlJess theorem. Let X be a locally compact space which
is countable at infinity and let S be a set in . "o(X). Then the following two
conditions are equivalent:
(i) S is relatively compact with respect to the vague topology.
(ii) S is equibounded, i.e.,
sup f I dJJ < 00 for all IE Co(X).
ES x
In addition, if X has a countable basis, then the vague topology on .Ao(X)
is metrizable, i.e., we have:
S is relatively compact = S is relatively sequentially compact.
In particular, we obtain the following result. Let X be a locally compact
space with a countable basis and let (IJ,,) be a sequence in .Jlo(X). Then the
following two conditions are equivalent:
(a) There exists a subsequence with JJ,,' vague. IJ as ,J -+ 00.
(b) sup" IS x j" dJl,,1 < 00 for all fEe o(X).
(88c) Weak. compactness theorem of Prohorov. Let X be a complete sepa-
rable mctric space (e.g., a separable B-space) and let (JJ,,) be a sequence in

The Sticltjes Integral
1081
...6 o (X). Then the following two conditions arc equivalent:
,,'eak ·
(i) There exists a subsequence with /JII' t p,. as n ..... oc.
(ii) sup" Jln(X) < oc and, for each t > 0, there exists a compact set C in X with
sup IJII(X - C) < t.
"
In addition, if X is only a separable metric space, then (ii) implies (i).
The Stieltjes Integral
(89) Ph}ts;cal motil'ation. Let J\I: IR -. R be a monotone increasing function
which is continuous from the left. We use the following physical interpretation:
M(b) = mass on the interval] -00, b[.
Hence if b > a, then
J\-/(b) - M(a) = mass on [a, b[.
In particular, we obtain that
M(a + 0) - M(a) = mass at the point a.
Here, we set J\1(a :t: 0) = lim M(b) as b -. a :t: O. For example. if there exists
exactly one mass point on R, say at a, then the mass distribution function M
has the form of Figure 13, where m is the mass of the point.
(90) Lebesglle-Stieltjes integral. According to (7Si), the function M gener-
ates a measure I' on R which is called a Stieltjes measure. The corresponding
integral
ffdP
is called a Lebesgue-Stieltjes integral.
(91) Tile classical Stieltjes integral for pie,.e,,'ise cOlJtinuous filnctions. In
order to prepare the definition of Stieltjes operator integrals which are impor-
tant for the spectral theory of self-adjoint operators, we recall the definition
of the classical Stieltjes integral which is a special case of the Lebesgue .
In
)
M
/
a
Figure 13

1082
Appendix
)-
f
. 

A
Figure 14
Stieltjes integral. In the following let the function
f: R--+C
be piecewise continuous, i.e., for all A, the one-sided limits f(A. + 0) exist and
each bounded open interval contains at most a finite number of points;' with
f(A + 0) =F f(A - 0) (Fig. 14). The basic idea of the following definition is the
relation:
L dM = mass on J
for all types of intervals J.
Definition.
Step I: Let f be continuous on the bounded open interval J = ]a, h[.
We define
f f dM = lim I "fdM.
J ,..,,+0 c
In this connection, the integral J f dM is defined as in Section 3.1 by the limit
of partial sums
I " ,.
f dM = lim L f(Ai)(M()t) - M().t-I )),
, "-'OCI i-I
where A, = c + k(b - c)/n. This limit exists according to a similar proof as in
Section 3.1.
Step 2: Let P = [a, a] be a point.
Then we define
Lf dM = f(a)(M(a + 0) - M(a)).
Step 3: Let J be an arbitrary bounded interval.
Then we use the disjoint decomposition
J = U J i + U t
i j
where f is continuous on the open interval lit and  denotes a point. We
define
f f dM = L f f dM + L f f dM.
J i J, j PJ

Spectra) Theory for Self-Adjoint Operators
1083
For example, we obtain
f f dM = f f dM + f f dM.
J (G.b( Jla.a) J )G.b{
Step 4: Finally, we define
f oo fdM = lim f fdM
- X) J ).J.bI
as a -+ -00 and b -. +00.
Spectral Theory for Self-Adjoint Operators
In the following let X denote an H-space over I< = lA, C.
(92) Basic ideas. Let A: R 2 -. (R2 be a symmetric matrix. Then there exists
a unitary matrix U: (R2 -. R 2 such that \\'e obtain the diagonalization (first
basic formula)
(92a)
( ) = UAU- I .
where ;.1 and ).2 arc the eigenvalues of A. Moreover, letting
_ -1 ( 1 0 )
PI - U 0 0 U.
_ -1 ( 0 0 )
P2 - U 0 I U,
we obtain the second basic formula
(92b) A = i". PI + ).2 P 2.
The goal of spectral theory is to generalize this classical result to self-
adjoint operators A: D(A) c X -+ X on H-spaces. The two main theorems are
the following:
(i) Diagonalization theorem of yon Neumann (102) (generalization of (92a».
(ii) Decomposition theorem of Hilbert (95) (generalization of (92b).
In this connection, formula (92b) is generalized to
A = f oo ldE;.o
-00
This way it is possible to construct functions f of A by means of the formula
f(A) = f_:c. oo f(,l)dEJ.'

1084
Appendix
This basic formula of the functional calculus generalizes the classical formula
-I ( /(A 1) 0 ) Ii · f 
f(A) = U 0 f(i'2) U = (I..)PI + (1. 2 )P 2 .
where A: 0i 2  R 2 is a symmetric matrix.
(93) By definition, a spectral falnil)t {E;.} on X is a family of orthogonal
projection operators E,t: X ..... X for all ,t e (R such that for all i p e R u e X
the follo\ving hold:
(i) E;.E" = EpEJ. = E" for J.l S A.
(ii) liml-'_ E,tu = 0 and lim A _ +(J.; EAfl = fl.
(iii) limA_,._o EAu = E"fl.
Consequently, if Jl  )., then
E..(X) C EA(X)
and E" = E A on EIJ{X).
(94) Slie/ljes operator integrals. For fixed u e X we set
J'" (;,,) = II E A U II :z.
Then the function M: R -+ R is monotone increasing and continuous from the
left. Let .f: R  K be piecewise continuous. Then the integral
f:f(l)dEAU
is defined completely similarly to the classical Stieltjes integral in (91). This
so-called operator integral exists iff
f_'XIX) If(A)1 2 d liE AU II 2 < 
holds in the sense of (91). By definition, the integrals of the type
f-: f(l)d(E"ulv)
arc reduced in a natural way to Stieltjes integrals with respect to M(A) =
II E  \V 11 2 by means of the identity
(EAfll v) = i( IIE(u + v) II 2 - II El(u - v)1I 2 - ; IIEA(u + ;v)II 2 + ill E.t(u - iv)1I 2 ).
In the case where X is real we set i = O.
(95) Tire first nUl;n theorem of spectral ,heor}' (Hilbert (l906a), v. Neumann
1929)). Let A: D(A) 5 X -+ X be a self-adjoint operator on the H-space X over

Functional Calculus for Self-Adjoint Operators
1085
IK. Then there exists exactly one spectral family {E;.} such that
(95a) Au = f-;x.). dE;.u for all U E D(A).
In this connection. U E D(A) iff the integral in (95a) exists, i.e..
r: ;.2 dllE;.ull 2 < 0Ci.
Conversely, each spectral family {E).} on X generates a self-adjoint operator
A on X by means of (95a).
Functional Calculus for Self-Adjoint Operators
(96a) D(init ion. Let A: D( A) c X -+ X be a self-adjoint operator on the
H-space X over (K and let f. g: R -+ IK be piecewise continuous functions. We
set
D(.f(A)) = {u E X: L: IJVWdIlE;.uI1 2 < :x:-J
and define the linear operator f(A): DCf(A)) e X..... X by the formula
f(A)!, = r f(A.)dE;.u for all U E D( (A».
Note that the right-hand integral exists iff II E DC((A)). Hence our definition
makes sense.
(96b) Properties of the calculus. In the following let B* denote the adjoint
operator to B.
(i) The operator f(A) is graph closed and densely defined. If f: R ...... K is
bounded, then the linear operator .((A): X -+ X is bounded with the
operator norm
II.r(A)11 < sup If())I.
). € R
(ii) The adjoint operator f(A)* corresponds to the conjugate complex func-
tion .r: R -. IK, i.e.,
f(A)*u = f_ex ]().) dE;.u
for all u E D(f(A)*)
with
D(f(A)*) = {u E X: L I fU. W dllE;.ulI2 < 00 }.
i.e., D(f(A)*} = D(f(A)).ln particular, if.r: IR -+ R is a real function, then
the operator f( A) is self-adjoint.

1086
Appendix
(Hi) For all u e D(f(A») and l' E X,
(f(A)ulv) = f: f(,t)d(E,tull1).
(iv) For f(l) = l-, n = 0, I, ..., we have
f(A) = A-
with AO = I. In particular, for all u E X,
U = fo2) dE).u, lIull 2 = f: dUE).uIl 2 .
(v) We have
f(A)g(A) = g(A).f(A) = (fg)(A).
Here, the bar denotes the closure of operators. If there exist positive
constants c and d with
Ig(,i)1 < clf().)g(i)1 + d
for all ). e R,
then
f(A)g(A) = (fg)(A).
(vi) The dense set  Define
y = U (Ell - E,t)(Ah
A<,.
where the union is taken over all indices i.., /J e R with A < Jl. Moreover,
let X/(A) denote the set D(f{A» equipped with the graph scalar product
(ulv)f(A) = (ulv) + (f{A)ul!(A)v).
Then f(A)(Y)  Y and the set Y is dense in the H-spaccs X and X/CA)'
For all U E Y:
f(A)g(A)u = g(A)f(A)u = (fg)(A)u.
(97) M ajorant criterion. Let J be an interval in R. Suppose that the function
f: R x J --. IK has the property that
;....... f()., t)
is piecewise continuous on R for all t E J. We set
f(A.t)u = f:o:. f(,t, t)dEAu for all u E D(f(A. t)).
By definition, u e D(f(A, t» iff
f_:l):l) If()., t)1 2 d II E). un 2 < 00.
Let U E n,eJ D(f(A, t») and let
x(t) = f(A, t)u.

Functional ('alculus for Self-Adjoint Operators
1087
Then:
(i) The function t t-+ x(t) is continuous on J provided there exists a piecewise
continuous function g: IR -+ R satisfying the following majorant condition
I/(;. 1)1  g{).) for all (A, t) E R x J,
and fx. g(A-)dIlE A uIl 2 < 00.
(ii) The function t t-+ .\"(t) is differentiable on J and we have
X'(l) = h(A, t)u
for all t E J
provided the derivative /,()., t) exists for all ()., I) E IR x J, the function
)......... f,().. t) is piecewise continuous on R for all I E J, and there exists a
piecewise continuous function h: R -. R satisfying the majorant condition
If,()., t)1  h(j.) for all (A, t) E R x J..
and f h(i)dIlEAUIf2 < 00. Here, we set
f,(A,t)u= f:f,().,t)dE"U.
(98) Generalization. Let A: D(A) c X --+ X be a self-adjoint operator on the
H-space X over Ik\ and let I: R -+ IK be a bounded Borel function in the sense
of (84). Then there exists exactly one linear continuous operator B: X  X
with
(Bu I u) = f  f().) d II E AU 11 2
This integral is to be understood in the sense of Lebesgue-Stieltjes (cf. (90)).
We set f(A) = B. Then
for all U EX.
I(A). = I(A)
and
IIf(A)11 < sup I/(A)I.
AE A
(99) Exanlple. Let A: 1R2 ..... (R2 be a real symmetric matrix with the eigen-
values ).1 < ;.2' Then there exists a unitary matrix U: n 2 --+ R 2 such that
A = U -1 ( ).I ? ) U.
o i. 2
We set
_ I ( I 0 )
PI = U 0 0 U.
Pz = U-I( )U.
Then the spectral family of A is given by
o
E;. = PI
PI + P 2 = I
for A < lIt
for A. I < ,{ < A. 2 ,
for )"2 < )".

1088
Appendix
We obtain
 = EAJ+O - E A ), j = I, 2.
Hence the formula Au = J ldEAu corresponds to
A = ).1 PI + A 2 P 2,
and the formula f(A)u = J f{)')dEAu corresponds to
 -J ( !(A I )
f(A) = f().1 )P 1 + f(1'o.2)P 2 = U 0
o ) u
!(A. 2 ) ·
(100) Standard exan1ple. Let A: D(A) c X --. X be a self-adjoint operator
on the separable H-space X over K and suppose that A has a complete
orthonormal system {u",} of eigenvectors with Au", = A",U"" m = I, 2, ... .
Additionally, we assume that inf", Am > -00. These assumptions are satisfied
if one of the following three conditions holds:
(i) The operator A: X --. X is linear and symmetric, and dim X < 00.
(ii) The operator A: X --. X is linear, symmetric, and compact.
(iii) The operator A is the Friedrichs extension of a linear symmetric and
strongly monotone operator and the embedding X£ c X is compact.
Here, X E denotes the energetic space of A (see Section 19.9).
The eigenvalues A", of A are counted according to their multiplicity. Let IJI'
JJ2' . · · denote the different eigenvalues of A, i.e., Pi :F PJ iff i =1= j. Moreover, let
Pic: X --. X denote the orthogonal projection operator onto the eigenspace
corresponding to the eigenvalue p" of A.
First suppose that JJI < JJ2 < ... and that there exists a largest eigenvalue
J-lma.. Then the spectral family of A is given by
0 for A.  Jll'
PI for III < A  1l2'
E A =
for Ilk < A.  p" + I ,
PI + ... + Pic
I JJma.. < l,
where k = 2, 3, .. . . In the general case, we define {E A } such that
E.. +0 - E1c = P"
for all k
and E-'L' = 0, E+ = I. Moreover, let E A = constant on )'-intervals which do
not contain eigenvalues of A. Finally, this definition has to be arranged in
such a way that E --. E A as JJ --. A - 0 for all A E R.
Then the formula Au = Jcx AdEAu is identical with the well-known
expansion
All = L A", ( u'" I u) u'" = L Jl" Pi U
1ft Ie
for all u E D(A).

Diagonahzatlon of Self-Adjoint Operators
1089
The formula f(A)u = Jx. f(A)dEAu is identical to
f(A)u = L f().m )(u m ' u)u m = L f(/J")P,,u for all U E D(f(A))
m "
and
D(f(A)) = {u E X: f-: IJ().)/2 dllE.t u lI2 < oc }
= {u EX:  IJ()'lftU 2 /(u lft lu)1 2 < Xl}.
Note that U E D(f(A)) iff the series L",f(A",)(ll m lu)u", converges.
Diagonalization of Self-Adjoint Operators
(101) I sonJetric and unitary operators. Let X and Y be H-spaces over IK = R,
C. A linear operator U: X -+ t' is called isometric iff
II U u II = II U II
forall ueX.
Such an operator is injective. Recall that U: X -+ Y is called u'Jitary or an
H-isomorphism iff U is linear, bijective, and
(UUIUI') = (ulv)
for all u, (' E X.
A linear operator U: X -+ X is unitary iff it is isometric and surjective.
(102) The second main theorem of spectral theory (v. Neumann (1949)). We
need the basic formula
(102a)
(A"'u)(m) = ;.(nJ)u(m)
for almost all m E M
and the commutative diagram
D(A) c: X
It. I
D(A) c: f
.4 . X
jll
A . f.
Let A: D(A) C X --t X be a self-adjoint operator on the separable complex
H-space X. Then:
(i) There exists a set M and a measure J.l on M with /J(M) < 00. Let f =
L(M,Jl), i.e., g consists of all measurable functions 12: M -+ C with
fM lul 2 dll < 00.
(ii) There exists a unitary operator U: X -+ f and a function ).: M -+ R which
is finite almost everywhere on M.

1090
Appendix
(iii) The operator U generates the transformation
14 = U u.
This way the operator A is transformed into the operator A = U A U -I.
(iv) The operator A has the simple form (I 02a) for all U E D(A) where
DCA) -= {u E g: AU E f}.
(v) U E D(A) iff Uu E D(A).
Renzark. This famous theorem shows that the H-space X can be realized by
the function space g = L(M,JJ). In this connection, the self-adjoint operator
A on X corresponds to the simple multiplication operator A. The set M may
be regarded as an index set for the generalized eigenvalues A.(m) of A. By the
classical Fourier transform, differentiation is transformed into multiplica-
tion. Hence the unitary operator U may be regarded as an abstract Fourier
transform.
(103) Exa,nple. Let A: (:2 -+ (:2 be a real symmetric or complex hermitian
matrix. i.e., a ij = a ji for all i, j, with the eigenvalues A( I), l(2). We set
A = C.I) ;.2»).
Then there exists a unitary matrix U: (:2 -+ C 2 such that
(IOJa) A = UAU- I .
We set X = e 2 , M = {1,2} and
( U( 1 ) ) = U ( U( 1 ) ) .
14(2) u(2)
Then (IOJa) corresponds to
(Au)(,n) = l(m)u(m)
for all m E M, U E C 2 .
This is the basic formula (I 02a) above. In fact, if we define the measure p on
M by JJ(m) = I for all m E M, then the space f = L(M, Jl) is equal to e 2 .
In this example we have g = X. Note that in the general case of theorem
(102) above we have to leave the space X, i.e., g  X.
Classification of the Spectrum of Self-Adjoint Operators
Let A: (RN --+ (RN be a symmetric matrix. Then all the eigenvalues of A are real
and the set of all the eigenvalues of A forms the spectrum a(A) of A. In the
case of general self-adjoint operators the structure of the spectrum can be
much more complex. This fact reflects mathematically the possibly complex
structure of the energy values of quantum systems (see (104<1».

Classification of the Spectrum of Self-Adjoint Operators
1091
(104) COlnplex spaces. Let A: D(A) c X -+ X be a self-adjoint operator on
the complex H-space X. The case of real spaces X will be considered in (105).
Let X =F {O}.
By definition, the point lEe belongs to the resoll'ent set p(A) of A iff
te inverse (A - )./)-1: X -+ X exists as a linear continuous operator. The
spectrum C1(A) of A is defined to be the complement of J,(A), i.e.,
C1(A) = C - p(A).
Here, O'(A) is a closed subset of R. We define the point spectrum of A by
C1 p (A) = set of all eigenvalues of A.
Moreover, we define the discrete spectrum of A by
O'di,c(A) = set of all eigenvalues of A of finite multiplicity.
(l04a) First classification of the spectrum C1(A) due to Hilbert. Our goal is
the not necessarily disjoint decomp ositio n
t1(A) = C1 p (A) u t1 c (A).
By definition, the operator A has a pure point spectrum iff the eigcnvectors of
A for m a complete orthonormal system in X. In this case, we have C1(A) =
t1 p (A), and we set O'c(A) = 0.
The operator A has a purely continuous spectrum in X iff A has no eigen-
values. In this case, we have C1 p (A) = 0, and we set C1 c (A) = O'(A).
We now consider the orthogonal decomposition
X = X pp Gj Xc'
"'here X pp denotes the closed linear hull of the set of all eigenvectors of A,
and Xc = X;p denotes the orthogonal complement to X pp. Then the opera-
tor 14 transforms the subspaces X pp and Xc into itself, and A has a pure point
spectrum on X pp and a purely continuous spectrum of on Xc. We define the
continuous spectruln of A by
O'c(A) = spectrum of A on Xc.
(l04b) Refined first classification of O'(A). Our goal is the disjoint decom-
position
C1 c (A) = O'ac(A) U usin.(A)
along with the orthogonal decomposition
Xc = X. C ffi X lin ..
To this end, for each fixed U E X, we set
M(A) = IIE A uII 2 .
By (90), the function M: (R --+ R generates a Stieltjes measure JlII on . We
defi ne:

1092
Appendix
(i) U E Xac; iff Pu is absolutely continuous with respect to the Lebesgue mea-
sure on IR.
(ii) u e X 1in , iff Pu is a continuous measure which is singular with respect to
the Lebesgue measure on R.
Moreover, we have:
(iii) II e X pp iff p. is a pure point measure.
The operator A transforms the subspaces X mc ' X sinl ' and X pp into itself. We
define the absolutely continuous spectrum a.(A) and the singular spectrum
G,inl(A) of A by
O'ac(A) = spectrum of A on X. c '
0'8in.(A) = spectrum of A on Xin8.
The corresponding definitions for measures may be found in (82).
(I04c) Second cIQssficatio'l o.f G(A). We define the essential spectrum of A
by
O'css(A) = Q'(A) - D'diK(A).
Thus. \\'e have the disjoint decomposition
a(A) = Q'dJ,c(A) U O'cM(A)
and (1di&c(A) C O'p(A) as well as O'c(A) c O'g(A). Let {E,.} denote the spectral
family of A. Then:
(i) The sets a(A) and Gcu(A) are closed subsets of R.
(ii) l e O'(A) iff {EtIf} is not constant on a neighborhood of Ar.
(iii) ;. e tJ'diK(A) iff 0 < dim(E,t+o - E,t)(X) < 00.
(iv) A e G,(A) iff dim(El+ - E;._)(X) = 00 for all t > o.
(v) ). e O'cls(A) iff there exists a Weyl sequence (u,,) for A, Le.,
AU n - )-11.. -+ 0
as n-+oo
and the bounded sequence (u,,) does not contain any convergent sub-
sequence.
(104d) PIJ}'sical interpretation. In quantum physics, each state of a quantum
system is described by a vector U E X in a complex H-space X normalized by
111111 = 1. The elJerg}' of the system is described by a self-adjoint operator
A: D(A) S; X --. X which is called the Hamiltonian of the system. The states
lleX pp and ueX
arc called bound and free, respectively. In particular, if
Au = ,wI
lIuli = 1,
then !I corresponds to a bound state of energy . Roughly spcaking if we
compare the electron oCthe hydrogen atom with a body in celestial mechanics,
then the bound and free states of the electron correspond to the orbits of
planets and freely moving comets, respectively.

Linear Semlgroups
1093
More generally, each physical quantity a of the system corresponds to a
self-adjoint operator A: D(A) c X -+ X (e.g., momentum, angular momen-
tum, etc.). Let {E A } be the spectral family of A. Let the system be in the stale
u and suppose that we measure the quantity a. It is typical for quantum physics,
that there exists a probability p(B) for finding the measured value of a in the
set B. This probability is given by the fundamental formula
p(B) = Is dM(i.),
where
M(l) = IIE,tull 2
for all A E R,
i.e., M represents the distribution function for the measured values of a, and
hence
M ()) = probability for finding a in the interval] - oc, A[.
In particular, if B is a subset of the resolvent set p(A), then p(B) = 0, and hence,
roughly speaking, only those values can be measured which lie in the spectrum
a(A). According to the theory of probability, the expectation value a and the
dispersion (l\a)2 for the quantity a are given by
a = f"x; i. dM ().),
(L\a)2 = f-: (A - a)2 dM(i.),
and hence
a = (Aulu),
(a)2 = ((A - al)2 1l I u ).
This underlines the fundamental importance of the spectrum and the spectral
family for quantum physics. We will study these questions in greater detail in
Chapter 89 of Part V.
(105) Real spaces. Let A: D(A) c X -+ X be a self-adjoint operator on the
real H-space X. By Problem 19.6, the complexification Ac is also a self-adjoint
operator on the complexified H-space Xc. By definition, all the spectral
properties of A refer to Ac. In particular, by definition, a(A) = a(A c ), etc.
Note that the spectral family of A( is equal to the complexification of the
spectral family of A.
Linear Semigroups
In the following we summarize basic results on linear semigroups in B-spaces.
This should help the reader to recognize important connections between the
linear and nonlinear theory of semigroups. For brevity, semigroups of linear
bounded operators on real or complex B-spaces are simply called linear
semigroups. The basic definitions on semigroups are contained in Section
19.17a.

1094
Appendix
( 106) Importance of generators. Two linear strongly continuous semi-
groups on a B-space are identical iff the generators are identical.
The generators of such semigroups are densely defined on the B-space and
graph closed.
(107) Main theorem on linear uniformly conti"uous semigroups. Precisely
all linear uniformly continuous semigroups .ff = {S(t)} on a B-space X are
obtained through
S(l) = e 'B
for all I > 0,
where B: X -+ X is an arbitrary linear bounded operator. Moreover, B is the
generator of Y.
If we consider e 'B for aliI E R, then we obtain one-parameter groups with
IIS(t)1I < eillUSIl for all t E fRo
Hence it is ilnpossible to describe typical irreversible processes in nature by
linear uniformly continuous semigroups. The generators of such processes
must be unbounded. This underlines the importance of unbounded operators
for the mathematical description of nature.
(108) Main theorem on lilJear strongly continuous semigroups (Hille Yosida
theorem). If {S(t)} is a linear strongly continuous semigroup on a B-space X,
then there exist real numbers p and M > I such that
(C)
IIS(I)1I < Melli
for all I > o.
The operator B: D(B) c X -+ X is the generator of a linear strongly con-
tinuous semigroup with (C) iff the following hold:
(i) B is linear, densely defined on X, and graph closed.
(ii) All real numbers )" > P belong to the resolvent set of Band
II(AI - B)-IIII  M(,t - fJ)-"
for all ,t > p,
n = I, 2, . . . .
The corresponding semigroup is obtained through
S(t)u = lim e '8 ,.u for all t > 0 and all u E X,
".--+0
where RIJ = (I - J-lB)-1 and the operator
BIJ = J-l- 1 (R". - I)
is called the Yosida approximation of B. We have B". = BR"..
The set of operators B with (i) and (ii) above is denoted by G(X, M, p).
With respect to (C), one has the following special cases:
p = 0: bounded semigroup;
M = I: quasi-nonexpansive (or quasi-contractive) semigroup;
M = It P = 0: nonexpansive (or contractive) semigroup.

Linear Semigroups
1095
(108a) The homogeneous Cauchy problem. Let BE G(X, M,Il) \\'ith the
corresponding semigroup {S(t)}. Then !l(t) = S(t)uo with Uo e D(B) is the
unique solution of the Cauchy problem
14'(1) = Bu(t),
o  t < oc,
u(O) = 1'0.
More precisely we have the following statements:
(IX) The map t  S(t)ll o is continuous from R+ to X for all 140 EX. Recall that
R+ = [0. ex>[.
(II) This map is differentiable at t = 0 (and equivalently. for all t  0) iff
U o e D(B). Moreover, if"o E D(B), then S(t)uo E D(B) for all t  0 and
d
dI 5 (I)Uo = 5(I)Buo = BS(t)r1o (or all t > O.
(it) Let X B denote the B-space D(B) equipped with the graph norm of B. Then,
for each 14 e X s, the mapping
t..-. S(t)u
is continuous from R+ to X B, and the mapping
d
1"- dt (S(I)U)
is continuous from R+ to X.
(108b) The inlw"wgeneous Cauch}. problem (strongl) conliIJuOIIS semi-
groups). Let 0 < T < 00. The Cauchy problem
14'(1) = BU(I) + J(t). 0 < t < 1:
u(O) = Uo
(P)
has a unique solution if the following conditions are satisfied:
(i) B generates a linear strongly continuous semigroup {S(t)} on the B-space
x.
(ii) "0 E D(B).
(iii) f: [0, T] -+ X is C 1 .
This solution is given by
(S) u(t) = S(t)u o + f Set - s)f(s) ds,
where fl E C( [0, T[, X)  C 1 ([0, T[, X) and
Il(t) E D(B) for all t e [0, T[.
(I08e) The in/,o".ogelleous Cauchy problem (anal)'t;c semigroflps). Let 0 <
1- < . The Cauchy problem (P) above has a unique solution if the following

1096
Appendix
conditions are satisfied:
(i*) The operator - B is sectorial on the complex B-space X.
(ii*) U o E X.
(iii*) f: [0, 1"] -. X is Holder continuous.
This solution is given by (5) above. Here,
u E C([O, T[, X) n C 1 (JO, T[, X),
and u(t) E D(B) for all t E ]0, T[.
Condition (i*) is equivalent to the fact that B generates a linear analytic
semigroup. Hence, (i*) is stronger than (i) in (I 08b). But observe that (ii*) and
(iii*) is weaker than (ii) and (iii), respectively.
(109) Characterization 0.( linear notJexpansive sem;groups. Let B: D(B) £;
X -. X be a densely defined linear operator on the B-space X. Then B is the
generator of a linear nonexpansive semigroup iff one of the following two
conditions is satisfied:
(i) - B is maximal accretive, Le., the inverse operators (I - pB)-I: X --+ X
exist for all p > 0 and arc noncxpansive.
(ii) B is maximal dissipative, i.e., for every u E D(B) there exists an element
u* E J(u) with
Re(u*, Bu)  0
and R(l - JJB) = X for some JJ > 0, where J: X -. 2 x . is the duality map
of X.
If X is an H-space, then condition (ii) is equivalent to
Re(uIBu)  0
and R(l - pB) = X for some Ji > o.
for all u E D(B}
Sectorial Operators, Analytic Semigroups, and
Parabolic Equations
(110) Let A: D(A) c X -. X be a sectorial operator on the complex B-space
X, i.e., the following conditions are satisfied:
(i) A is linear, graph closed, and densely defined on X.
(ii) There are numbers C E H, M  I, and y E ]0, 7t/2[ such that the open sector
1: = {l E C: y < larg(A - c)1 < n, =F c}
is a subset of the resolvent set of A and
II(I - A)-III  MI). - cl- I
for all  E 1:.
Then - A is the generator of a linear analytic semigroup {e- 1A }, which is

Seclonal Operalors, Analylic Semlgroups. and Parabolic Equ3110ns
1097
/ ,
/ , --
,I' , -
 
.. y( ,-
'" '/
, 'c -c /
 /
j , /
I , / -
r -[
(a) (0)
H
/
r
-- /c
.
(c)
Figure 15
given by the formula
e -/ A = -  f. ()./ + A) - 1 e A' d;..
21t1 c
Here, C is a smooth curve in -1: with arg).. -+ + (n - r) as Ii. I -+ oc (Figs.
15(a), (b)). Note that e-. A is independent of the concrete shape of C.
A sectorial operator with c = 0 is called strictly sectorial.
(1IOa) Standard exanzple I. Let A: D(A) c X  X be a linear self-adjoint
operator on the complex B-space X with
(Aulu) > c(ulu)
for all u E D(A) and fixed C E R.
Then ,4 is sectorial, and -.4 generates a linear analytic semigroup {e -'A}.
If, in addition, c = 0, i.e., A is monotone, then A is strictly sectorial, and
{e-'A} is both a linear bounded analytic semigroup and a nonexpansive
semigroup. More precisely, we have
lIe-,AII < 1
for all tee \\'ith Re t > o.
(I lOb) Standard example 2. Let A: D(A) c X -. X be a linear, graph closed,
and densely defined operator on the complex B-space X. Suppose that there
is a real number c such that the closed half-space
H = {; E C: Re;.. < c}
is a subset of the resolvent set of A (Fig. 15(c)) and
const
II(A - nrlll < 1 + l,tl
for all ;. E H.

1098
Appendix
Then, the operator A is sectorial with respect to 1: in (110) for some
y E ]0, 1[/2[, and hence - A generates a linear analytic sem;group. This follows
from Problem 1.7.
(1IOc) Standard example 3 (elliptic differential operators and parabolic: equa-
tions). We consider the parabolic differential equation of order 2m:
u, + Au = 0
on G x ]0,00[,
(I)
Byu(x, t) = 0 on cG x ]O,oo[
u(X, 0) = uo(x) on G,
where Byu = OY u and
for a II }': I y I  m - I,
Au = L (- I )IClI DCI( a ClJl OJI u).
ItJl m
We assume:
(i) G is a bounded region in R N with iJG E Ca. I , where N, m > I.
(ii) The differential operator A is strongly elliptic, where all the coefficient
functions aClJl: G -+ R are COO.
We set
x = Lp(G),
Let D(A} be the closure of the set
{u E COO(G): Byu = 0 on aG for all y: Iyl < m - I}
in the Sobolev space W p 2 m(G). Then the operator
I < p < 00.
A:O(A) c X-+X
(II)
is well defined in the sense of generalized derivatives. Moreover, it is of
great importance that the operator A satisfies all the conditions of Standard
example 2 in (II Ob) if we consider the complexification of A. This fact has the
following fundamental consequence.
The original problem (I) can be written in the form:
u'(t) + Au(t) = 0, 0 < t < 00,
u(O) = U o .
Let {S(t)} be the analytic semigroup generated by - A. Then, for each U o E
D(A), the unique solution of (II) is given by
(III) u(t) = S(t)u o .
If Uo EX, then we regard u(.) in (III) as a generalized solution of (II) and (I).
This important result can be extended to general classes of boundary
conditions (cf. Friedman (1969, M), Theorem 19.4}. This way it is possible to
investigate general classes of parabolic equations (and parabolic systems) via
the theory of sernigroups.

Fractional Powers of Sectorial Operators
1099
(1IOd) Character;zatio'l of linear bounded analytic semigroups. Let X be a
complex B-space. We define the open sector
1:(1 = {z E C: larg zl < (x, z  O}.
By a linear bounded analytic semigroup {5(t)} we understand the following:
(a) {S(t)} is a linear analytic scmigroup on r:l for fixed  E ]0, 1[/2[.
This means that the operator S(t): X --+ X is linear and continuous for each
I E r, and the mapping I  5(1) is analytic from rcr into L(X, X). Moreover,
S(O) = J and S(t + s) = 5(t)5(5) for aliI, S E rcr. Finally, S(t)u --+ u as t --+ 0 in
r 2 , for each U E X.
(b) For each p with 0 < {J < (X,
sup 115(t)1I < ;xJ.
, E I,
If the operator - A is the generator of such a linear bounded analytic
semigroup, then the spectrum O"(A) is contained in the closure of the sector
I:.lf/2)-a.
More precisely, the following two statements are equivalent:
(i) The operator A: D(A) c X -+ X is slriclly sectorial on the complex 8-
space x.
(ii) The operator - A is the generator of a linear bou'lded analytic semigroup.
(llOe) Characterization of linear analytic sem;groups. The following two
statements are equivalent:
(i) The operator A: D(A) c X --+ X is sectorial on the complex B-space X.
(ii) The operator - A is the generator of a linear analytic semigroup.
Fractional Powers of Sectorial Operators
(III) Let the operator A: D(A) £; X -+ X be sectorial as in (110), where X
is a complex B-space with X :F {O}. We set
B = A + aJ
and choose the real number a in such a way that Re 0"(8) > 0, i.e., Re O"(A) +
a > 0, where u(A) denotes the spectrum of A.
For every (X > 0, we define
I f. 
B-« = f(Ot) 0 lll-Ie-,Bdl.
Then B-tJ: X --+ X is linear, bounded, and injective. Moreover, we set
Ir = (8- a )-1
for all (X > 0,
and 8 0 = I.

1100
Appendix
For all Ct, fJ > 0, these fractional powers have the following properties:
(i) Ir is densely defined on X and graph closed.
(ii) If 0    p, then D(BP) c D(/r), and
IrBP = BPIr = /r+/l on D(Ir+/l)
as well as B-ClB-/l = B-/lB- = B-(2-11 on X.
(iii) e- 1A maps X into D(B) for aliI> o.
(iv) For all u E D(Jr) and I > 0,
Ire-1Au = e- 1A /ru.
(v) For all U E D(B CI ) and 0 < a < I,
IIIrull > IIB-II-Illuli.
Therefore, the set X(J = D(Ir), equipped with the graph norm
lIull(J = lIB"ull,
becomes a B-space (abstract Sobolev space).
If 0  Ct  (1, then the embedding
(E) X/l c X
is continuous, and X/l is dense in X(I. For Ct = 0, we get Xo = X.
If 0  Ct < P and if the operator A has compact resolvent, i.e., the
operator (A.I - A)-I: X ..... X is compact for each A. in the resolvent set
p(A), then the embedding (E) is compa(.t.
(vi) For all u E D(B) and 0  Ct  I,
II B CI u II < co n s t II Bull Clil u 111 -  .
In terms of the norm 11.11 2 on XCI' this is the interpolation inequality
lIul/(J < const lIull Ilull -(I for all u E XI.
(vii) Suppose that Re a(A) >  > o. Then there are constants K and M
depending on Ct such that
II A (J e - ,''' II < K I - CI e - dl, 0  ex < 00,
lI(e- I '" - I)ull < Mt(JIIAull,
for all t > 0 and u E D(A CI ).
Suppose that Re a(A) > b with b E R. Then there is a constant K such that,
for all t > 0,
o < a < I,
II A e - I A II  K t - 1 e - dt,
II e - I It II  K e - dt .
If we consider the special linear operator A: X ..... X, where X = C and
A > 0 is real, then the fractional power A(J in the sense above coincides with
the classical notion for each real cx.
Further important properties of fractional powers can be found in Henry
(1981, L) and Pazy (1983, M).

Interpolation Theory In B-Spaces
) ) 01
Interpolation Theory in B-Spaces
The basic idea of interpolation theory is contained in the following diagram:
Jl T A.
.
[V, H]o T · [X, y]o
11 1 Y, 0< 8 < I.
t
For given linear continuous operators T: V -+ X and T: H --+ Y between
B-spaces, we want to construct new B-spaces [V, H]o and LX, Y]8 by Uinter-
polation" such that the operator T remaillS cont;n'IOUS.
In this connection, we will describe the following important interpolation
methods:
(i) the real method (K-method) for B-spaces;
(ii) the complex method for B-spaces; and
(iii) the interpolation between H -spaces by letting
[V, H]o = D(B 1 0),
i.e.. this method uses fractional powers of monotone self-adjoint operators
B.
We shall show below that methods (i) and (ii) yield different results for
the Sobolev spaces Wp'"(G) if p # 2. Method (iii) is a special case of (i) and (ii).
The following two estimates are of fundamental importance for modern
analysis. Let". 110 denote the norm on the interpolation space [H]o. Then
we shall obtain estimates of the form
" II "8 < con s t "U" :' - 811 u 111,
for all U E V n 1/
and
/I Tile < \I TII  -oil TII,
where we set 1
[ V, fI]/I = {
for 0 = 0
for 0 = 1.
Here. the operator norm 111118 refers to the corresponding interpolation spaces.
The classical prototype of interpolation theory is given by the famous
Convexity Theorem of Marcel Riesz (1926) for Lp(G)-spaces which will be
considered in (113) below. The general interpolation theory was created
around 1960. The complex method was introduced independently by A.
Calderon.. S. Krein, and J. Lions. The K-method is due to J. Pcetre.
There is the following philosophy behind interpolation theory. For example,
I This definllion is ver) natural Ho\\'ever, nOle thai there also exist different definitions in Ihe
hterature which are, roughly speakIng, based on continuity properties of [V, H]. as 0 -.0 and
o  I Herc.l J'.II], denotes an arbitrary interpolation method.

1102
Appendix
consider a differential equation briefly denoted by
Tu = f.
In order to study this concrete analytical problem via functional analysis, we
regard.T as an operator between certain function spaces. However, the point
is that there are many different choices for the function spaces. Interpolation
theory helps us to study systematically the properties of T in different spaces.
In particular, interpolation theory may help us to find "natural" spaces A and
B for T, i.e., the operator T: A --+ B is a homeomorphism. In fact, such natural
results for linear elliptic partial differential equations may be found in Lions
and Magenes (1968, M), and in Triehel (1978, M), (1983, M).
(112) The K-method for B-spaces. By definition, an interpolation couple
{X, Y} consists of two B-spaces X and Y over IK = R, C. Moreover, in order
to have the sum X + Y at hand, we assume that there is a topological vector
space Z such that both the embeddings
X £; Z and Y £; Z
are continuous. For Z E X + Y and t E R, we set
K(z,t) = inf {"xlix + tIlYllr}.
z=.x+,
Herc, the infimum is takcn over all possible decompositions z = x + y with
x E X and Y E Y. For 0 < 0 < I, we define
(f.o '"' K (z, 1)1- 1 - 8, dl ) 1/' for I < r < 00,
IlzlI,.r =
sup K (z, l)t-' for r = 00.
o < , < .x.
Now our basic dejlnition reads as follows:
[X, Y],., = {z E X + Y: IIzlI,., < oo}.
Here, 0 < 0 < I and I  r  00.
The following four theorems are important.
(a) B-space. The set [X, Y],., together with the norm 11'11,., becomes a
B-space over K with
X n Y c [X, Y],., c X + Y
and
IIzlI s .,  c,.,lIzlI -9I1zll
where C S . r denotes a constant.
(b) Density. Lct 0 < 6 < I and I < r < 00. Then the set X (""\ Y is dense in
[X, y]o.,'
for all z E X n Y,
(c) Linear continuous operators. Let 0 < 0 < 1, I  r  00, and let {V, H}

Standard Examples for the Interpolation of Function Spaces
1103
and {X, Y} be interpolation couples over (K. Moreover, let
T: V + H -+ ..\' + Y
be a linear operator and suppose that the two restrictions
T: V-+X,
T: H --+ Y
are linear continuous operators with the corresponding norms II TII 0 and
II Till' Then the restriction
T: [V, H]e., -+ [.,t, Y]e.,
is also a linear operator with the norm II Tile., and
II Tile.,. < IITII-9IfT".
(d) Duality. Let 0 < (J < I, 1 < p < 00, and p-l + q-l = I. Suppose that
X n ): is dense both in X and Y. Then
([X, Y]e.p)* = [X*, Y*]8.q'
Let X, Y, and Z be B-spaces. By convention, a relation of the form
[X, Y].. = Z
below means that the set [X, Y] is equal to the set Z, and the corresponding
norms are equivalent. The same convention will also be used for a relation of
the form X = z.
Standard Examples for the Interpolation of
Function Spaces
(113) Lebesgue spaces (Convexity Theorem of M. Riesz (1926)). Let G be a
nonempty measurable set in R N with N > I (e.g., G is open or closed). Let
I < p, q < 00,0< 0 < I, and
r - I = (I - 8) p - 1 + 8q -1 .
Then
[Lp(G), Lq(G)]s.,. = L,.(G)
and from the Holder inequality it follows that
lIull, < lIu" -Situ II: for all U E Lp(G) n Lq(G).
Suppose that the operator
(113a) T: L,( G) -+ L ll ( G)
is linear for all I  p, q < 00. Let C be the set of all points (p-I, q -I) in R 2 for
which the operator (113a) is continuous and denote the corresponding opera-

1104
Appendix
tor norm by II TII P.f' Then the set C is convex and the function
(p-l, q-I ).......In II Tit,.,
is convex on C. This follows from (112c).
(114) Holder splices. Let G be a bounded region in R N with oG e c and
ly > I. For r = 0, I, ... and 0 <.2 < 1, \ve set
Then
- -
C'f':I(G) = C,.cr(G).
[erc( G ). C"'( G )],. X) = CJ( G )
for real numbers 0  k < j < m < 00 and
j=(1-O)k+8m,
o < 8 < 1,
wherej is not an integer. In the special case where k = 0, nl = 1, and 0 < 8 < 1,
we obtain
[C(G), C1(G)],.  = CI( G ).
(115) Sobolev spaces (real method). Let I < p < 00. Under the same
assumptions as in (114 we obtain that
(I) [W,I;(G Wp"(G)]o.P = Wl(G).
This relation remains valid for G = R'.
If p = 2, thenj is also allowed to be an integer.
(116) Sobolet' spaces (colnplex method). Let I < p < 00 and let k, j, m be
ilJlegers. Under the same assumptions as in (114), \ve obtain that
(II) [W;(G), W,:"(G)], = Wl(G).
This relation remains valid for G = R N .
Here, [ ., · ], corresponds to the so-called complex method whose definition
can be found in (1100) below. For many applications it is sufficient to know
that the fundamental theorems (a)-(d) in (112) remain valid for the complex
,netlJod if \ve use complex B-spaces and if we assume, in the dualit)' theorem
(d), that X or Y is reflexive.
In the special case where k = 0, In = 2, 1 < p < 00. and 0 < 8 < J t we obtain
that
[L,,(G), W p 2 (G)],." = W,,2D(G)
if 8 #- 1/2 and
[L,,(G), W p 2 (G)] 1/2 = Wpl(G).
Furthermore, we have
[L 2 (G), W Z 2 (G)]'.2 = W 2 2 '(G), 0 < 0 < 1.
(116a) All importallt special case. Let p = 2. If G = R'Y, l\r > 1, then the

Standard Examples lor the Interpolation of Function Spaces
1105
relations (I) and (II) above remain true for all real numbers k, m, 0 with
-00 < k < m < 00
and
o < 0 < I.
If G is a bounded region in R N with smooth boundary. i.e., oG E Cr%, then
(I) and (II) remain true if 0 S; k < na < 00 and 0 < 8 < I.
All these relations above remain valid if \\'C replace the real Sobolev spaces
by the corresponding complex Sobolev spaces.
(116b) The ,(uPldamental role played b)7 Besov spaces and Triebel- Lizorkin
spaces. Let the bounded or unbounded region G be given as in (1168) above.
If p =# 2, then the interpolation of So bole v spaces may lead to lIe' spaces. The
prototype of this fact is given by the relation
[Wpt(G)c, W,"'(G)c],.q = B.q(G).
\vhere 0  k < m < 00, 1 < p, q < 00,0< 8 < 1, andj = (1 - O)k + O,n.
More precisely, for -00 < nl < 00 and 1 S p, q s 00, it is possible to define
the spaces
(S) ,(G) and Fq(G).
\\'hich are called Besov spaces and Tr;ebel-L;zork;n spaces. respectively. The
fairly simple definition via Fourier transform will be given in (116d) below.
(In the case of the spaces f;:q, the index p = oc is excluded).
All the spaces (5) are complex B-spaces whose elements are distributions.
The point is that:
(a) important classical function spaces are special cases of (S), and
(P) the spaces (S) possess extremely elegant interpolation properties, in con-
trast to Sobolev spaces.
Moreover, the spaces (S) play a fundamental role in the theory of linear
elliptic differential equations as discussed in Problem 22.10.
Standard exal1lple 1 (Sobolev spaces). Let -OCJ < m < oc;, I < p < 00. and
G = RN. Then
W"' ( G\ = { F;2(G)
p 1(: r ( G )
p.p
If G is bounded, then this relation remains true provided m 'I: -en + p-l).
n = O. I.  . . . .
Standard example 2 (Holder spaces). Let m = 0. 1,2,... and 0 < a < I. Then
C"'.CZ( G) c = S::(G),
where the functions f e C-.«( G) c are complex-valued.
Example 3 (Bessel potentials). For -cc < m < oc,. and I < p < 00, one also
uses the notation
if m = 0, :t 1, :t 2, . . . ,
otherwisc.
H';(G)c = F: 2 (G).
The elements of these spaces are called Bessel potentials.

1106
Appendix
Example 4. For -00 < m < 00 and 1 < p < 00,
B;'.p(G) = F;p(G).
Moreover, if -00 < m < 00 and G = R N , then
W 2 "'(G)r = H';(G)r = B'2'.2(G) = f"2.2(G).
This relation remains true if G is bounded provided m :F -1, - , - i, . . . .
(116c) I"he .{uIJdamental interpolation theorem. Let the bounded or un-
bounded region G be given as in (116a). Let -00 < k < m < 00,0 < 0 < 1,
and
j = (I - O)k + Om
as well as 1  PO,PI,P,qO,ql,q < 00 with
1 (1 - 6) 0
-=- +-,
P Po PI
I (I - 0) 0
-= +- ,
q qo ql
where we use the convention "1/00 = 0". Then the K-method and the complex
interpolation method yield the following fundamental relations:
(A) [B;."o(G), B".(G)]s." = Bt.q(G),
(B) [F;."o(G), F;". (G)]8." = Bt.q(G),
(C) [B:o.qo(G), B;..4l.(G)]s = Bt.q(G),
(D) [F:o."o(G), F;:.", (G)]8 = Ft.,,(G).
Here, we exclude P = 00 in (B) and P = 00, q = 00 in (D).
Relations (I) and (I I) in (115) and (116) above are special cases of (A)-(D)
because of (116b).
The proofs, along with further important properties of these spaces, can be
found in Triehel (1978, M), (1983, M) (e.g., density, embedding, duality, and
generalized boundary values). This theory is essentially based on the Fourier
transform and on so-called Fourier multipliers.
(116d) The basic de!;'lit ions.
Step I: Let G = R N .
The Besov space B:,,(R N ) consists precisely of all the tempered distributions
U E !/' ({RN) with
( B) 1112 "" F - I CPt Full p I q < 00.
Similarly, the Tr;ebel-Lizork;n space F;q(G) is obtained if we replace the
condition (B) with
(F) 1112"" F -I CPt Ful,,1I p < 00.
The spaces B;,,(G) and F;,,(G) become complex B-spaces under the norms
(B) and (F), respectively.

Standard Examples for the Interpolation of Function Spaces
1107
We now explain our notation. As usual, F denotes the Fourier transform.
Moreover, {cp,,} is a fixed system of functions
<PI: E 9'(R N ), k = O. I, _ _ -.
which forms a partition of unity, i.e.,

L l/\(x) = 1
t=o
for all . e 11I''1,
and lJ'o(x) * 0 implies I..I  2 as well as
tp.(x)  0 implies 2 A - 1  Ixl  2'+1
for k = I, 2, ... . In addition, for each multi-index 
sup 2' lcal ID-<Pt(x)1 < 00_
".x
Finally, II-III' denotes the usual norm on the Lebesgue space Lp(R.'l), and, for
brevity of notation, we set
la t l 4 =
CO ,a k , 4 r'4
sup" I at I
if I  q < 00,
if q = 00.
Note that the functions FlPt.F- 1 u are analytic on IR''l for U E g>'(IR''l). If we
change the system {CfJt}, then the norms (B) and (F) above are replaced by
equivalent norms.
Step 2: Let G be a bounded region in R N with aG e C(£ .
By definition. 14 E q(G) iff u e !i}'(G, C) and
(R) 14 = V on G for some v e B;:q(IIIN).
Then, B;:q(G) becomes a complex B-space under the norm
null = inf {n vllr (AN.},
I' "..
where the infimum is taken over all v e B;:.(R N ) which have the restriction
property (R).
Analogously, we obtain the space F::.(G).
These definitions are due to Triebel (1973).
(116c) Definition oJ'the conlplex interpolatioPl method. We define the open
st ri p
s = {z e C: 0 < Rez < I}.
and, for j = 0, 1, we set
ajs = {zeC: Rez =j}.
Then as = iJoS v i\ S. Let X and Y be two complex B-spaces which form an

1108
Appendix
interpolation couple. Let 0 < lJ < I. By definition,
U E [X, Y]o
iff
U = /(O),
where f: S -+ X + Y is some function which has the following properties:
(i) .r is analytic on S;
(ii) the restrictions f: ooS -+ X and f: ill S -+ Yare continuous, and the sets
j'(OjS) are bounded for j = 0, I.
In this connection, we equip X + Y with the norm
IIwll = inf {lIxli x + IIYllr},
w::.x+y
for all \" E X + Y, where the infimum is taken over all the decompositions
w = x + y with x E X and Y E Y.
Then [X, Y]o becomes a complex B-space under the norm
lIuli o = inf {lIfll}.
/(8) = II
where the infimum is taken over all the functions f which have the properties
(i), (ii). and we set
IIfll = max L,s II/(z) II x. :s II/(z) II y }.
If X and Yare real B-spaces, then we define
[X, Y]s = [Xl' Yc]s n (X + Y),
where X c and Y( denote the complexification of X and Y, respectively (cf.
A I (23h)).
(116f) Monotone self-adjo;'11 operators and interpolation theory. Let
B: D(B) £; X -+ X
be a linear monotone self-adjoint operator on the H-space X over IK = A, C.
Let 0 < . p < 00 and
y = (I - (J) + Op,
where 0 < 0 < 1. We set
X 2 = D(Ir).
Then, X becomes an H-space over IK under the graph scalar product
(U I v)a = (u Iv) + (Ber u I Jr' v).
By the K-method and the complex method,
[X a , X p ]O.2 = [XCI' Xp]o = X'I"

H-Spaces. Fractional Powers, and the Friedrichs Extension
1109
Interpolation Between H-Spaces, Fractional Powers,
and the Friedrichs Extension
(117) De/in;t ion. Let
"V c H c v. n
be an evolution triple where V and Hare H-spaces. We construct a linear
operator A: D(A) £; H -+ H by the relation
(U"')J-' = (Au I v)" for all u E D(A). I' E H.
To be precise, let D(A) be the set of all u E H such that the map v (ull')11 is
linear and continuous on H. Let u E D(A). By the Riesz theorem, there exists
a '" E H such that
( U II' ) V = (", I () II
for all I' E H.
Now let Au = "'.
The operator A is self-adjoint and we have
(Aulu)" = IIull:. > c lIull;, for all U E D(A) and fixed c > o.
Define B = A I 2 and the "graph scalar producf.
(UII')8 = (B 1 -sui B 1 -0(,)" for all u, v E D(B 1 -8).
Now our basic definition reads as follows:
[V,H]8 = D(B I - 8 ).
(118) Basic properties. The following four theorems are important.
(a) H-space. Let 0 < () < I. Then the set [V. H]s, together with the scalar
product ('1' )s. becomes an H-space. For all ( E V,
IIvll s < Ilvll -8111'11.
Moreover, we obtain
v C [V.H]8 C H
and [V,H]o = V. [V,H]I = H.
(b) Density. The set V is dense in [V, H]9 for all 0  0 < I.
(c) Linear continuous operators. Let "V c H c v. n and ux c y c X.'. be
two evolution triples. Let
T: II -+ Y
be a linear continuous operator and suppose that the restriction
T: V -+ X
is continuous. Then all the restrictions
T: [V,H]9 -+ [X. Y]8.
o < 0 < I.
are continuous.

1110
Appendix
(d) [It: V*]1/2 = H.
By ourdetinition of an evolution triple"V S H  v*" given in Section 23.4,
the spaces V and H arc real. However, the same method above also applies
to the complex case, i.e., we may assume that V and H are separable H-spaces
over 0< = (R, C such that V is dense in H and the embedding V S H is
continuous. By (116f), the interpolation method above coincides with both
the K-method [., · ]2.' and the complex method.
(119) Standard exanJple. Let H be a real separable H-space and let A:
D(A) c H  H be the Friedrichs extension of a linear, symmetric, strongly
monotone operator. We set
v = Hf:,
i.e., V is the energetic space of A. Let us assume that the embedding HE S; H
is compact. Then the operator A has a complete orthonormal system of
eigenvectors (un) in H with Au" = 2..u". Moreover, we obtain
[  II], = D(B 1 -0).
\"here B = A 1.'2, and II e [V, H], iff
( ) I
II ull" = ,i -'l(u.1 u)1 2 < 00.
Special case. Let G be a bounded region in 1Ji''', N  1. We set H = L 2 (G).
Let A denote the Friedrichs extension of the negative Laplacian
-: CO=(G) -+ H.
Then HE = W 2 1 (G).
Application to Sobolev Spaces
(120) Standard e.anJple. Let -00 < k < m < 00 and j = (I - O)k + 8m
\\'ith 0 < 8 < 1. Let G = R.", N > I. Then
(R) [Wl(G), W 2 "'(G)], = WJ(G)
Now let G be a bounded region in HI'I with smooth boundary, i.e., cG e C.
Let k, ''', andj be given as above. If, in addition, k, m, andj are different from
the critical values -1, -!, -}, .... then relation (R) above remains true.
Moreover, we get
[W;(G), W 2 "'(G)], = Wj(G)
provided 0 S k < m < 00 and k, m, and j are different from the critical values
1 .a1 
2' 2' 2' ... ·
All these relations above remain valid if we replace the real Sobolcv spaces
by the corresponding complex Sobolcv spaces.

Hausdorff Measure.. Hausdorff Dimension.. and Fractals
1 I 1 I
(121) The trace theorem. Let "V s; H s; V." be an evolution triple. We set
iI'2 = {u E L 2 (0, T; V): U(III) e L 2 (0, T; H)},
where nJ = I, 2. ... and 0 < T < 00. This set becomes an H-space with the
scalar product
(ul v) = (Ill V)Ll(O. T: V) + (u(lft) I V(III)Ll(O. TIH).
Let II e /2M. Then, for j = 0, 1, ..., m - I, the derivatives have the following
properties:
IlJ) E L 2 (O, T; [V, H]J'M)'
flu) e C( [0, T], [H]U.1/2)l"").
The so-called trace map
u t-+ (u(O), u'(O). . . , U(_-1 )(0»
is surjective from 1I. to nj.-J [V, H]u+II2),....
Hausdorff Measure, Hausdorff Dimension, and Fractals
The following notions play an important role in the modem regularity theory
for partial diffcrential equations (partial regularity), and in the modern theory
of evolution equations and dynamical systems (e.g., the Navier-Stokcs equa-
tions). We recommend Federer (1969, M), Giaquinta (1983, M), Simon (1983,
L) Giusti (1984 M), Temam (1983, M), (1988, M), and Mandelbrot (1982. M)
(fractals).
( 122) Hausdorff measure. Let X be a metric space (c.g. t X is a subset of A" ).
Let 0  m < 00 and b > O. By definition, the m-dimensional Hausdorff mea-
sure of thc noncmpty set X is given by
( I 22a)
where
H""(X) = lim H;'(X).
...o
H:'(X) = infL (2- 1 diam Bi)M,
.
the infimum being taken over all at most countable coverings (B i ) of the set
X by subsets Bt with
diam B i  b for all i.
If such a covering of X does not exist, then we set H:'(X) = 00.
The number Hi(x) is called the outer 6-Hausdorff measure of X. The limit
in (122a) is well defined since (H;'(X» is monotone increasing as  -. 0, and
hence
H'"(X) = sup Hi(X).
1>0
Notc that 0 S H-(X)  co. For m = 0, we use the convention "0- = I".

1112
Appendix
Consequently, if X consists of a single point, then HO(X) = 1 and H-(X) = 0
for all n, > O.
If the set X is empty, then we define H"(0) = H;(0) = 0 for all m > 0 and
tS > o.
The number
Jf'm(x) = V",H"'(X)
is called the normalized Hausdorff measure of X, where
v. = nt)"'/r( ; + I).
for all In  o.
In particular, if m = 1, 2, . . . , then V", denotes the volume of the n,-dimensional
unit ball in R"'.
E_amples. Let m = I, 2, .... If X is an m-dimensional ball in R"', then
the normalized m-dimcnsional Hausdorff measure JY"'(X) is identical to the
classical volume of X.
More generally, we have
Jf)m(x) = meas X
for all subsets X of R'" which arc measurable with respect to the m-dimensional
Lebesgue measurc. This Lebesgue measure is denoted by meas X. For arbi-
trary subsets X ofR"', r(X) is identical to the outer m-dimensional Lebesgue
measure J and H"'(X) = H;(X) for all  > o.
If X denotes a sufficiently smooth m-dimensional surface in IRa", l.J > m, then
Jf''''(X) = classical surface measure.
More precisely, this relation holds if X is an nJ-dimensional C1-submanifold
of R N , ft., > m.
For m = 0, we get
HO(X) = J('o(X) = { card X
+00
if X is a finite set,
otherwise,
whcre card X denotes the number of distinct points of X.
The proofs can be found in Simon (1983, L).
Note that there exist different definitions of the Hausdorff measure in the
literature. For example, if we replace the arbitrary subsets B i of X with closed
balls 8 in X, then H;(X) and H"'(X) in (122a) are replaced with S;(X)
and S'"(X), respectively. Here, S'"(X) is called the m-dimensional spherical
Hausdorff measure of X. Similarly, 9''"(X) == V",S'"(X) is called, the normal-
ized ",-dimensional spherical Hausdorff measurc. Obviously,
H'"(X) < sm(x) and Jr"'(X)  g''"(X) for all m > o.
Ho\vcver, note that there exist pathological subsets X of R". such that
H"'(X) :#= S"'(X) for some nJ, and hence JY"'(X)  '"(X).

Func.ions of Rounded Variation
1113
(123) II arlsdorff dinlension. The Hausdorff dimension of the metric space X
in (t 22) above is defined to be
dim H X = inf{I1J > 0: H-(X) = O}.
Roughly speaking, sets with a low Hausdorff dimension are "small... If
H'"(X) = 0 for some nJ, then dim H X S m.
In particular, H-(0) = 0 for all m  0 and hence dimH 0 = o.
One can sho\v that
Hm(X) = { o if nJ > dim H X,
+00 if nJ < dim H X,
i.e., the Hausdorff dimension dim H X corresponds to a "phase transition" of
the Hausdorff measure H"'(X) of X.
If dimH X < 00, then X is homeomorphic to a subset of some R' v .
(123a) E.anlple. Let X be an at most countable subset of a metric space,
and let n be the number of distinct points in X. i.e.. 0  n  00. Then
H"'(X) = { o r m > 0,
" If m = o.
Hence dimH X = o.
(124) Fractals. A metric space is called a fractal iff its Hausdorff dimension
strictly exceeds its topological dimension.
The notion of the topological dimension can be found in Definition 13.15.
(124a) Example. Let X be the Cantor ternary set, i.e., X is the collection of
all real numbers represented by the formula
C 1 C2 c 3
Z = jT + 3 2 + 3) + .. · ,
where the coefficients c. arbitrarily assume one of the two values 0 or 2. Then
dim H X = log 2/1og 3 = 0.6309. . .,
whereas the topological dimension of X is equal to zero, i.e., X is a fractal.
Furthermore, the set X has the one-dimensional Lebesgue measure zero.
This example shows that the Lebesgue measure is not always the appro-
priate tool for measuring the size of sets.
Functions of Bounded Variation
Functions of bounded variation play a fundamental role in the modern
calculus of variations. In what follows, the two ke}t results are given by

1114
Appendix
(i) the general integration by parts formula; and
(ii) the general existence theorem for parametric minimal surfaces.
(125) Basic defilJilion. Let G be a nonempt}' open set in fRN, N  1. The
function u: G -. R is called a function of botl,uled variation iff 14 e L. (G) and
there exists a real constant K > 0 such that
(125a) f J. UDitp. dx  K max 1 cp(x)1
i-I G xcG
for all CPl,.'.' CPr-' E Co(G), where Icp(x)1 denotes the Euclidean norm of cp(x) =
(<PI ().. · · . <PN(X», The smaUest possible constant K  0 in (125a) is denoted
by JG IDui t i.e., we set
fG IDul = inf K.
Let BV(G) denote the space of all the functions 14: G -. R of bounded
variation. Then, BV(G) becomes a real B-space under the norm
lIullBVCGI = lIull,_I{G) + fG IDul.
Morc generally, the definition of JG IDIII also makes sense if 14 e LI.lo(G).
Finally. we set JG IDul = 00 iff there is no real K > 0 such that inequality (125a)
holds truc. Summarizing, we have
fG IDul = sup fG udiv rpdx ,
where the supremum is taken over all the functions <p e C6'(G, RI) \vith
1q>(x)1  I on G. As usual, we set div cp = rr-l DiCPj.
(126) Standard example 1. Let u e C 1 ( G ), where G is a nonempty bounded
open set in R 1t ', N > I. Then, u E BV(G) and
(126a) fG IDul = fG 1 grad ul dx,
where I grad ul = (r'7-1 (D i u)2)112.
Proof. For all tfJl' .. ., CPr-' e CO'(G), integration by parts yields
J. r-' J. N
r UD.tpi dx = r (,O,D,udx
G j-l G '_I
S fG I rp (x)ll grad u(x)1 dx
< (J. I grad ul dX ) max 1q>(x)l.
G .EG

Functions of Bounded Vanation
1115
Hence JG IDIII < fG I grad ul d:(. An additional argument shows that JG IDul <
JG I grad uldx is impossible. 0
More generally, if G is a nonempty bounded open set in (Rho, N  1,
then
Wtl(G)  BV(G),
and relation (126a) holds true for all u e W p l (G), 1 < p < 00.
(127) Standard example 2. Let E be a bounded region in R', N  1,
with smooth boundary, i.e., oE E C z . Let Xl: be the characteristic function of
E. i.e.,
XE(X) = {
Then \\'C get the fundame,atal formula
(127a) f, IDlEI = surface measure of the boulldary oE.
R
More generally, if G is a nonempty open set in R''', then
fG IDXEI = surface meaSilre of the set aE ("\ G.
In the case where the boundary oE is not sufficiently smooth, we call
IR'" IDlEI the generalized (N - l)-dimensional surface measure of aE.
if x e E,
if x e (Ji.aJ - E.
(128) Generalized derivatives. Let U E BV(G). It follows from (125a) via the
Riesz theorem that there exist a measure p on the set G and p-measurable
functions i: G -+ R, i = I, ..., N, such that
(I 28a) fG uD(q>dx = - fG q>a.jdp for all q> e Cg>(G).
and i = 1, .. ., N. In particular, if G is a bounded region in R'v and u E C. ( G 
then II e BV(G) and
f. uDttpdx = - f. cpD,udx for all If) e CO'(G).
G G
Hence formula (128a) holds with
«t = Dt u and IJ = Lebesgue measure.
In terms of the theory of distributions, relation (128a) tells us the following:
TIre partial derivative D.u of the function u e BV(G) corresponds to the
measure .2; dJl.
In fact, let U denote the distribution corresponding to the function u e BV(G),

1116
Appendix
I.e.,
U(q» = fG u(/)dx for all (/) e C'cf(G).
Then, DiU(cp) = - U(Dilp) for all lp e Co(G). By (I 28a),
D;U«(/) = fG (/)rl;dp for all (/) e CO'(G).
(129) The general integration by parts formula. Let G be a bounded region
in (RN, N > I, with oG e CO. I. Then, for each function u e BV(G), there exists
a function U o e L. (aG) such that
(129a) J. UDitp dx = - J. tpa.i dp + J. uO<pni dO
G G G
for all qJ E CO'(R N ) and; = I, ..., N. Here. n = (n 1 ,..., ,I N ) denotes the outer
unit normal to the boundary aG.
Obviously, this is a generalization of the classical integration by parts
formula
J. IlD,fP dx = - J. cpD,1l dx + J. Uo<P". dO,
G G cG
where Uo = 14 on aG.
(130) Compacr"ess. Let G be a nonempty bounded open set in (RIt., N > 1.
Then the embedding
BV(G) C LI (G)
is compact.
(131) SemicolltinuilJ'. Let G be a nonempty bounded open set in R N , N > 1,
and let (14ft) be a sequence in BV(G) such that
U II .... u in LI (G) as n -. 00.
Then
J. IDul  lim J. JDu"l.
G IIOO G
(132) Caccioppoli sets. A subset E of R. is called a Caccioppoli set iff E is
a Borel set and XE E BV(G). i.e..
J. IDlEI < 00,
G
for every nonempty bOIl,uled open set G in R N .
(133) The ge"eral existence theorem for parametric mininJal surfaces. We

References to the Literature
1117
consider the following minimum problem:
r IDXEI = mint. E is a measurable set in R N ,
J H'"
E = C outside G.
( 133a)
Let G be a given bounded open set in R N , N  I, and let C be a given
Caccioppoli set in R'v.
TheIl the IJJi,JiIJU4IJJ problem (133a) IJas a 501'41ion Eo.
Interpretation. Recall that fR'IDlEI denotes the generalized (N - 1)-
dimensional surface measure of the boundary oE of the set E. Thus, roughly
speaking, the boundary aE o of the solution Eo of problem (133a) minimizes
the area among all surfaces with boundary ac " aGe
The simple proof of this theorem via compactness (130) and semicontinuity
(131) can be found in Giusti (1984. M). This monograph also contains sophis-
ticated results about the regl41aril)1 of the set oE o as well as applications of
the space BV(G) to the classi cal nonpara metric minimal surface problem:
fG  I + u: + u: dx = mint.
u = 9 on cG..
where :< = (t ,,).
References to the Literature
Function spaces: Kufner, John, and Fuik (1977, M) (introduction). Tricbel (1978. M).
(1983, M).
Sobolev spaces: Neau (1967, M) and Kufner, John, and Fucik (1977. M) (intro-
duction), Adams (1975, M) (standard work), Lions and Magenes (1968. M), Friedman
(1969, M) (inequalities ofGagliardo-Nirenberg) Triebel (1972, M), (1978. M). (1983.
M) (Sobolev spaces and interpolation theory), Nikolskii (1975, M), Mazja (1985, M)
(sharp embedding theorems), Wloka (1982, M) (Fourier transform and Sobolev
spaces
Weighted Sobolev spaces and partial dilTerential equations: Kufner (1985, M).
Kufner and S8ndig (1987, M).
Sobolev spaces of infinite order and dilTerential equations: Dubinskii (1984, L).
Sobole\' spaces on manifolds: Aubin (198 M), Eichhorn (1988) (cf. also the
References to the Literature for Chapter 85).
Measure theory and integration: Lebesgue (1902) (classical work), Halmos (1954.
M Kamke (1960. M), He\\'itt and Stromberg (1965, M), Bauer (1968, M) (applications
to the theory of probability. measures on topological spaces), Dicudonne (1968, M),
Vol. 2, Gunther (1972, M), Vol. 3, ROgel and Tasche (1974, M Mukherjea and Potho-
ven (1978, M Royden (1988, M).
History of the Lebesgue integral: Hawkins (1970, M
Geometric measure theol)': Federer (1969. M), Simon (1983, L).
Functions of bound cd variation and minimal surfaces: Simon (1983, L), Giusti (1984,
M).

1118
Appendix
Hausdorff measure, Hausdorff dimension, and partial regularity of the solutions of
partial differential equations: Giaquinta (1983, M), Simon, (1983. L), Giusti (1984, M),
Temam (1983, M), (1988, M).
Fractals and their applications: Mandelbrot (1982, M).
Radon measures on topological spaces: Schwartz ( 1973, L).
Vector measures: Dinculeanu (1966, M Holmes (1975, M).
Integration of functions with values in B-spaces: Dunford and Schwartz (1958, M),
Y osida (1965, M), Bagel and Tasche (1974, M).
Distributions: Kanwal (1983, M) (elementary introduction with many applications),
Schwartz (1950, M) (classical standard work), Gelfand and Silov (1964, M), Vols. 1-5,
Y osida ( 1965, M), Horvath ( 1966, M), Reed and Simon (1971, M ), Vol. I (introduction),
Vladimirov (1971, M).
Distributions and partial differential equations: Hormander (1964, M), (1983, M),
V ols. 1 4.
Spectral theory of unbounded operators: Riesz and Nagy (1956, M), Dunford and
Schwartz (1958, M), Vols. 1-3, Kato (1966, M), Maurin (1967, M), Triehel (1972, M)
(functional calculus).
Spectral theory and applications in quantum theory: Reed and Simon (1971, M),
V ols. I -4 (standard work), Triehel (1972, M).
Linear semigroups: Davies (1980, M), Pazy (1983, M).
Sectorial operators and analytic semigroups: Henry (1981, L).
Interpolation theory: Reed and Simon (1971, M), Vol. 2 (introduction), Lions and
Magcnes (1968. M), Vol. I. Bergh and Lofstrom (1976, M), Triehel (1978, M) (compre-
hensive representation), Krein, Petunin, and Semenov (1982, M).
Interpolation theory and semigroups: Butzer and Berens (1967, M).
Interpolation theory and partial differential equations: Lions and Magenes (1968,
M), Triebel (1978, M), (1983. M).
Galerkin schemes: Ciarlet, Schultz and Varga (1969), Michlin (1969, M), (1976, M),
Aubin (1972, M), Meis and Marcowitz (1978, L).
Finite elements: Ciarlet (1977, M) (standard work), Zhimal (1968), Strang and -ix
(1973, M), Bathe and Wilson (1976, M), Akin (1982, M), Babuka and Szabo (1988,
M), Ciarlet and Lions (1988, M), Vol. 2 (handbook of numerical analysis).
Software for finite elements: Sewell (1985, M).
Nonlinear differential equations and finite elements: Temam (1977, M) and Girault
and Raviart (1986, M) (Navier-Stokes equations), Frehse (1977), (1982, S), fe.rehse and
Rannacher (1978), Dobrowolski (1980), Dobrowolski and Rannacher (1980), Thomee
(1984, M), Kufner and Sandig (1987, M) (weighted Sobolev spaces), Feistaucr and
enikk (1988).

References
Ablo\\'itz, M. and Segur, H. (1981): Solitons alld the Inl'erse Scattering Trall.iform.
SIAM, Philadelphia, PA.
Ablowitz, M., Bcals, R.. and Tenenblat, K. (1986): On the solution o./the generalized
",'ave and generali:ed sine- Gordon equations. Stud. Appl. Math. 74, 177 203.
Adams. R. ( 1975): Sobolel' Spaces. Academic Press, New York.
Agmon, S., Douglis. A., and Nirenberg, l. (1959): Estimates near the boundar}' for
solutions of elliptic partial differential equations, I. II. Comm. Pure Appl. Math. 12
(1959),623-727; 17 (1964),35-92.
Agmon, S. (1965): Lectures on Elliptic Boundary Value Problems. Van Nostrand,
Princeton, NJ.
Akin. J. (1982): Application and Implementation of Finite Elemellt Methods. Academic
Press, New York.
Albcverio, S. et al. (1986): Nonstandard Methods in Stochastic Anal.'sis and Mathe-
matical Physics. Academic Press, New York.
Albrecht. P. (1979): Die numerische Behandlung ge'ohn/icher Differentialgleichungen.
Akademie- Verlag, Berlin.
Aleksandrov, P. [ed.] (1971): Die Hilbertschen Prohleme. Geest & Portig, Leipzig.
All, H. and Luckhaus, S. (1983): Quasilinear e/lipt;c- parabolic equations. Math. Z. 183,
311 - 341.
AIt, H. (1985): Lineare Funktionalanalysis. Springer-Verlag, Berlin.
AIt, H. and Struwe, M. (1989): Nonlinear Functional Analysis and Variational Problems
(monograph to appear).
Altman. M. (1986): A Unified Theory of Nonlinear Operator and Et'olution Equatiolls
Iilh Applications. Dekker, New York.
Amann, H. (1969): Ein Existenz- und Eindeuligkeitssatz fUr die Hammersteinsche
Gleichung in Banachriiumen. Math. Z. III. 175- 190.
Amann. H. (1969a): Zum Galerkin- Verfahren fUr die Hammersteinsche Gleichung. Arch.
Rational Mech. Anal. 35, 114- 121.
1119

1120
References
Amann, H. (1972): Existence theorems for equations of Ilammerstein type. Applicable
Anal. I, 385 - 397.
Amann, H. (1972a): 0 ber die lJiiherungsK'eise Lsung nichtlinearer I ntegralgleichungen.
Numer. Math. 19. 19- 45.
Amann, H. (1976): f';xed-point problems and nonlinear eigenvalue problems in ordered
Bunach spaces. SIAM Rev. 18, 620 709.
Amann, H. (1984): E.istence and regularity for semi/i"ear parabolic elJolut;on equations.
Ann. Scuola Norm. Sup. Pisa, CI. Sci. Serie I V, II, 593 -- 676.
Amann, H. (1985): Global existence for semi linear parabolic sysrems. J. Reine. Angew.
Math. 360, 47 - 83.
Amann, H. (1986): Quasi/inear evolution equations and parabolic systems. Trans. Amer.
Math. Soc. 293, 191- 227.
Amann, H. (1986a): Quasi/inear parabolic systems under nonlinear boundary conditions.
Arch. Rational Mech. Anal. 92, 153 - 192.
Amann, H. (1986b): Semigroups and nonlinear evolution equations. Linear Algebra Appl.
84, 3 32.
Amann, H. (1986c): Parabolic evolution equations with nonlinear boundary conditions.
In: Browder, F. [ed.] (1986), Part I, pp. 17-27.
Amann, H. (1987): On abstract parabolic fundalnental solutions. J. Math. Soc. Japan
39, 93- 116.
Amann, H. (1988): Remarks on quasilinear parabolic systems (to appear).
Amann, H. (1988a): Dynamic theory of quasilinear parabolic equations, I, II (to appear).
Amann, H. (1988b): Parabolic evolution equations in interpolation and extrapolation
spaces. J. Funct. Anal. 78, 233-277.
Amann, H. (1988c): Parabolic equations and nonlinear boundary conditions. J.
Differential Equations 72, 201-269.
Ambrosetti, A. and Prodi, G. (1972): On the inversion of some differentiable mappings
with singularities between Bana('h spaces. Ann. Mat. Pura Appl. 93, 231 - 246.
Ambrosetti, A. and Rabinowitz, P. (1973): Dual variational methods in critical point
theory and applications. J. 'unct. Anal. 14, 349 - 381.
Ambrosetti, A. (1983): Existence and multiplicity for some classes of nonlinear problems.
In: Warsaw (1983), Vol. 2, pp. 1125-1132.
Ames, W. (1965): Nonlillear Partial Differential Equations in Engineering. Vols. I, 2.
Academic Press, New York.
Ames, W. (1977): Numerical Methods for Partial Differential Equations. Academic
Press, New York.
Anselone, P. (1971): Collectively Compact Operator Approximation Theory. Prentice-
Hall, Englewood Cliffs, NJ.
Ansorge, R. (1978): Differenzenapproximation partieller Anfangswertaufgaben. Teubner,
Stuttgart.
Appell, J. (1987): The superposition operator (Nemyckii operator) in function spaces: A
survey. Mathematical Institute, Report No. 141, University of Augsburg.
Arms, J., Marsden, J., and Moncrief, V. (1982): The structure of the space of solutions
of Einstein's equations. II: Several Killing fields and the Einstein- Yang - Mills
equations. Ann. Physics 144, 81-106.
Atiyah, M. and Hitchin, N. (1988): The Geolnetry and Dynamics of Magnetic Mono-
poles. University Press, Princeton, NJ.
Attouch, H. (1984): Variational Convergence for FunClionals and Operators. Pitman,
London.

References
1121
Aubin, J. (1961): Behal,ior of the error of the appro:(;mate solution of boundary l'alue
prob/ms jor linear elliptic operators. Ann. Scuola Norm. Sup. Pisa CI. Sci. 21,
599-631.
Aubin, J. (1910): Approximation des problemes au:( Iimites non homogenes pour des
operateurs non Iineaires. J. Math. Anal. Appl. 30, 510- 521.
Aubin, J. (1972): Appro_timation of Elliptic Boundary Value Problems. Wiley. New York.
Aubin. T. (1982): Nonlinear Analysis on Manifolds: Monge -Ampere Equations.
Springer- Verlag. New York.
Babic, V. et al. (1967): Lineare Differentialgleichungen der Malhematischen Physik.
Akademie- Verlag, Berlin.
Babin, A. and Visik. M. (1983): Regular attractors of semigroups and evolution equations.
J. Math. Pures Appl. 62.441 -491.
Babin, A. and Viik, M. (1983a): Attractors of evolution equations and estimates for
their dimensions. Uspekhi Mat. Nauk 38 (4), 133 -187 (Russian).
Babin. A. and Visik. M. (1986): Unstable int'ariant sets of semigroups oj nonlinear
operators and their perturbations. Uspekhi Mat. Nauk 41 (4), 3 - 34 (Russian).
Babuska, I. and Szabo, B. (1988): Finite Element Methods: Concepts and Applications.
Springer-Verlag, New York.
Bahri, A. and Coron. J. (1988): On a nonlinear elliptic equation: the effect of the topolog}'
on the domain. Comm. Pure Appl. Math. 41, 253 294.
Ba'ire. R. (1899): Sur les fonct;ons des variables reelles. Ann. di Mat. 3. I -123.
Ballman, J. and Jeltsch. R. [eds.] (1988): II }'perbolic: Problems. Lecture Notes in
Mathematics, Springer- Verlag, Berlin (Proceedings to appear).
Banach. S. and Steinhaus, H. (1927): Sur Ie principe de condensation des sinyularites.
Fund. Math. 9, 50- 61.
Banach, S. ( 1929): Sur les fonct ;onelles linea ires. St udia Math. I, 211 - 216. 223 - 229.
Banach. S. (1932): Theorie des operations linea ires. Warszawa.
Bannerjee. P. and Butterfield. R. (1981): Boundary Element Methods in Engineering
Sciences. McGraw-Hili. London.
Barbu. V. (1976): Nonlinear Se,nigroups and Differential Equations in Banach Spaces.
NoordhofT. Leyden.
Bardos. C. (1982): Introduction aux problemes hyperbolique non Iinea;res. Lecture Notes
in Mathematics, Vol. 1047. Springer- Verlag, Berlin, pp. 1-76.
Bardos. C.. Degond, P.. and Golse, F. (1986): A priori estimates and existence results
for the Vlasol' and Boltzmann equations. Lectures in Applied Mathematics. Vol.
23, Amer. Math. Soc., Providence, RI, pp. 189-201.
Bathe. K. and Wilson. E. (1916): Numerical Methods in Finite Element Analysis.
Prentice-Hall, Englewood Cliffs, NJ.
Bauer, H. (1968): Wahrscheinlichkeitstheorie und Grundzuge der Mafttheorie. De
Gruyter. Berlin.
Bauer. F., Garabedian, P.. and Korn, D. (1972/77): A Theory of Supercrilical Wing
Sections, ,,'ith Computer Examples, Vols. 1-3. Springer-Verlag. New York:
Rauer, F.. Betancourt, 0., Garabedian, P. (1918): A Computational t.fethod in Plasma
Ph."s;cs. Springer- Verlag. New York.
Bauer. F., Betancourt. 0., and Garabedian. P. (1984): MagnelohydrodYllamic Equilib-
rium and Stability of Stellarators. Springer- Verlag, New York.
Bauer. F.. Betancourt, 0., and Garabedian. P. (1986): Alonte Carlo calculation of

1122
References
transport for three-dilnensional magnetohydrodyna,nic equilibria. Comm. Pure
Appl. Math. 39, 595-621.
Bazley, N. and Weinacht, R. (1983): .4 class of operator equations in nonli,Jear
mechanics. Applicable Anal. 15, 7 -14.
Hazley, N. and Miletta, P. (1986): Forced Litnard equations with nonlocoal nonlinearities.
Applicable Anal. 21, 339-346.
Bebernes, J. and Eberly, D. (1988): A description of self-similar blow-up for dimension
n  3. Ann. Inst. Henri Poincare, Analyse Non Lineaire 5, 1-21.
Beckert. H. (1976): Variatiollsrechnuny und Stabilitiitstheorie. Math. Nachr. 75,47-59.
Beckert, H. (1984): Nichtlineare Elastizitiitstheorie. Rer. Sachs. Akad. Wiss. Leipzig.
Math.-nat. KI. 117, 2. Akademie- Verlag, Berlin.
Belleni-Morante, A. (1979): Applied Semigroups and Evo/r41ion Equat;olas. Clarendon,
Oxford.
Benilan, P., Crandall, M., and Pazy, A. (1989): N olalinear f;volut ion Governed by
Accretive Operators (monograph to appear).
Benkert, F. (1985): ()ber den globalen Losungszusammenhang einiger Scharen 1J0n
Variatiolasproblemen. Dissertation, Karl-Marx-Universitiit, leipzig.
Benkert, F. (1989): On the number of stable local nlilaima of some functionals. Z. Anal.
Anwendungen (to appear).
Bensoussan, A., Lions, J. t and Papanicolaou, G. (1978): As}'mptotic Methods ill Periodic
Structures. North-Holland, Amsterdam.
Berezin, F. and Shubin, M. (1983): The Scohrodinger Equation. University Press,
Moscow (Russian).
Berger, M. and Podolak, E. (1974): On nonlinear Fredholln operator equations. Bull.
Amer. Math. Soc. BO, 861-864.
Berger, M. and Podolak, E. (1975): On the solutions of a nonlinear Dirichlet problem.
Indiana Univ. Math. J. 24, 837 -846.
Berger, M. (1977): Nonlinearity and Functional Analysis. Academic Press, New York.
Berger, M. and Schechter, M. (1977): On the solvability of :iemilinear gradient operator
equatiotls. Adv. in Math. 25, 97 132.
Berger, M. (1979): Non/itlear problems with exactl}' three solutions. Indiana Univ. Math.
J. 28, 689 -698.
Berger, M. and Church, P. (1979): Complete integrability and perturbation of a nonlinear
Dirichlet problem. Indiana Univ. Math. J. 28, 935-952; 29, 715 - 735; JO, 799.
Berger, M., Church, P., and Timourian, J. (1985): Folds and cusps in Banach spacoes witla
applications to nonlinear differe,uial equations. Indiana Univ. Math. J. 34, 1-19.
Berger, M. and Kohn, R. (1988): A resealing algorithm for the numerical calculation (r
blo\ving-up solutions. Comm. Pure Appl. Math. 41, 848-863.
Bergh, J. and Lofstrom, J. (1976): Interpolation Spaces. Springer-Verlag, New York.
Berkeley (1983): Nonlinear Functional Analysis. Cf. Browder, F. [cd.] (1986).
Berkeley (1986): Proceedings of the International Conference of Mathematicians ill
Berkeley. Vols. 1,2. Amer. Math. Soc., Providence, RI.
Berndt, W. (1982): Solution of a degenerated elliptic equation of second order ill an
unbounded domain. Z. Anal. Anwendungen I (3), 53 68.
Bers, L.t John, F., and Schechter, M. (1964): Partial Differential Equations. Wiley, New
York.
Besov, 0., IIjin, V., and Nikolskii, S. (1975): Integral Representations of Functiono" and
Embedding Theorems. Nauka, Moscow (Russian).
Betancourt, o. (1988): Betas, a spectral code for three-dimensional magnetohydro-

References
t 123
d)"Jamic equilihrium and nonlinear .-.tahility calculations. Comm. Pure Appl. Math.
41. 551 - 568.
BirkhofT, G. [ed.] (1973): A Source Book in Classical Analysis. Harvard University
Press, Cambridge, MA.
BirkhofT, G. and lynch, R. (1984): Numerical Solution o.f Elliptic Prohlems. SIAM,
Philadelphia, P A.
BlrkhofT. G. and Schoenstadt, A. [eds.] (1984): Elliptic Problem Solvers. Academic
Press, New York.
BirkhofT, G. (1989): 1'he softK'are systems ELLPACK and 11'PACK (to appear).
Blanchard, P. and Bruning, E. (1982): Direkte Methoden der Variationsrechnung.
Springer- Verlag, Wien.
Blaschke, W. (1942): Nicht-Euklidische Geometrie und Mechanik. Teubner, Leipzig.
Blaschke, W. (1957): Reden und Reisen eines Geometers. VerI. der Wiss.. Berlin.
Blumenthal, O. (1932): Lebensgeschichre von H Uberl. I n: Hilbert. D. ( 1932). Vol. 3.
pp. 388 429.
Bobenko, A. and Bordag, L. (1987): The qualitat;l'e analysis of multi-c'loidal K'aves (r
the Korteweg-de Vries equation via automorphic functions. Zapiski Naucnych
Seminarov LOM I, Vol. 165, pp. 31-41. Nauka, Leningrad (Russian). (English
translation in J. Sov. Math. (to appear).)
Bobcnko. A.. Its. A.. and Matveev. V. (1991): Anal}1tic Theory of Solitons. Nauka.
Moscow (Russian) tmonograph to appear).
Boffi, V. and Neunzert. H. [eds.] (1984): Applications of Mathematics in Technology.
Teubner, Stuttgart.
Bogel, K. and Tasche, M. (1974): Analysis in. norm;erle'l Riiulnen. Akademie- Verlag,
Berlin.
Bohm, M. and Showalter. R. (1985): A nonlinear pseudoparabolic diffusion equation.
SIA M J. Math. Anal. 16, 980-999.
Bohme, R. (1972): Die IJosung der Verz"'eigungsgleichungen fUr nichtlilleare Eigen"'ert-
probleme. Math. Z. 127, 105-126.
Bohmer. K. and Stetter, H. [eds.] (1984): Defect Correction Alethods: Theor}' and
Applications. Springer- Verlag, New York.
Bona. J. and Smith, R. (1975): Tire inil ial-value prohlem for the K orte\\'eg - de Vr;e.
equation. Philos. Trans. Roy. Soc. London A278. 555-601.
Bona, J. and Scott. R. (1976): Solution of the Korteweg-de Vr;e. equation in fractional
order Sobolet' spaces. Duke Math. J. 43, 87 -99.
Bonsall. f". and Duncan, J. (1971): Numerical Range of Operators of Normed Spaces
and of Elements of Normed Algebras. University Press, Cambridge, England.
Bony, J. (1981): Calcul symho/ique el propagation des singularitts pour les equations au:c
der;I'ees part;elles non Iinea;res. Ann. Sci. Ecole Norm. Sup. 14, 209 - 246.
Bony, J. (1984): Propagation et interaction des singularites pour les solutions des equa-
tions aux derivees partielles non lineaires.ln: Warsaw (1983), Vol. 2, pp. 1133-1147.
BOOS5, B. and Bleecker, D. (1985): Topolog}' and Analysis. Springer- Verlag, New
York.
Borisovic, Ju. et al. (1977): Nonlinear Fredholm maps and the Leray-Schauder theory.
Uspekhi Mat. Nauk 32 (4), 3 54 (Russian).
Botha. J. and Pindler, G. (1983): Fundamental Concepts in the Numerical Solutions of
Differential Equations. Wiley, New York.
Bottazzini, U. (1986): The "Higher Calculus". A History of Real and Complex Analysis
from Euler to Weierstrass. Springer- Verlag, New York.

1124
References
Bourbaki, N. (1940): Topologie genera Ie. Hermann, Paris.
Bourbaki, N. (1952): Integration. Hermann, Paris.
Braess, D. (1986): NOldinear Approximation Theory. Springer-Verlag, New York.
Bramble, J. and Osborne, J. (1973): Rate of convergence estimates for non-self-adjoint
eigenl'alue approximations. Math. Compo 27, 525 .549.
Brandt, A. (1982): Guide to multigrid development. In: ljackbusch, W. and Trottenberg,
u. [eds.] (1982), pp. 220- 312.
Bratley, P., Bennett, L., and Schrage, L. (1987): A Guide to Simulation. Springer- Verlag.
New York.
Brebbia, C. et al. (1984): Boundary Elenlent Techniques: Theory and Applications in
Engineering. Springer-Verlag, New York.
Brezis, H. (1968): Equations et inequations non linea ires dans les espaces vectorie/s en
dualite. Ann. Inst. Fourier 18. 115 - 175.
Brezis, H. and Sibony, M. (1968): Methodes d'approximation et d'iterat;on pour les
operateurs mOlJotones. Arch. Rational Mech. Anal. 28, 59 -82.
Brezis, H. ( 1970): On sonle degenerat e parabolic equat ions. I n: Browder, F. [ed.] ( I 97Oc),
pp. 28 - 38.
Brezis, H., Crandall, M., and Pazy, A. (1970): Perturbations of maximal monotone sets.
Comm. Pure Appl. Math. 23,123-144.
Brezis, H. and Pazy, A. (1970): Accretive sets a'ld differential equations;1I Banach spaces.
Israel J. Math. 8,367-383.
Brezis, H. (1973): Operateurs maximaux monotones. North-Holland, Amsterdam.
Brezis, H. and Browder, 1-.. (1975): Nonlinear integral equations and systems of H ammer-
stein type. Adv. in Math. 18, 115 144.
Brezis, H. and Haraux, A. (1976): Image d'une somme d'operateurs monotones et
application.... Israel J. Math. 23, 165-186.
Brezis, 1-1. and Browder, F. (1978): Linear maximal monotone operators and singular
no,Jlinear integral equations of Hamnrerstein type. In: Cesari, L. [ed.] (1978),
pp. 31-42.
Brezis, H. and Nirenberg, L. (1978): Characterization of the ranges of some nonlinear
operators and applications to boulldary I'alue problems. Ann. Scuola Norm. Sup.
Pisa CI. Sci., Ser. IV, 5, 225-326.
Brczis, H. and Nirenberg, L. (1978a): Forced vibrations for a nonlinear wat'e equation.
Comm. Pure Appl. Math. 31, 11-30.
Brezis, H. and Lions, J. (1979): Nonlinear P DEs and Their A ppli(:at ions. College de
f"rance Seminar. Vols. 1-8 (to be continued).
Brezis, H., Coron, J., and Nirenberg, l. (1980): Free vibrations for a nonlinear wave
equatio" and a theorem of P. Rabinowitz. Comm. Pure Appl. Math. 33,667-689.
Brezis, H. (1983): Analyse fonctionelle. Masson. Paris.
Brezis, H. and Nirenberg, L. (1983): Positive solutions of nonlinear elliptic equations
involving critical Sobolev exponents. Comm. Pure Appl. Math. 36, 437 -477.
Brezis, H. and Coron, J. (1984): Multiple solutions of H-systelns and Rellich's ("'onje('ture.
Comm. Pure Appl. Math. 37, 149- 187.
Brezis, H. (1986): Elliptic equations with limiting Sobolev exponents: the impact of
topology. Comm. Pure Appl. Math. 39, Supplement S 17 -S39.
Brezis, H., Crandall, M., and Kappel, F. [eds.] (1986): Semigroups and Applications,
Vols. I, 2. Wiley, New York.
Brelis, H. ( 1987): Liquid crystals and energy e.'itimates for S2 -valued maps. I n: Ericksen,
J. and Kinderlehrer. D. [eds.] (1987), pp. 31-52.

References
1125
Brezzi, F. (1986): N e.... application... of mixed finite element methods. I n: Berkeley (1986),
Vol. 2, pp. 1335-1347.
Brouwer, L. (1912): 0 ber Abbildungen von M annigfaltigkeiten. Math. Ann. 71, 97 -1 t 5.
Browder, F. (1954): Strong!.}' elliptic sJ'stems of differential equations. In: Bers, L. [ed.].
C"ontrihutions to the Theory of Partial Differential Equations, pp. 14-52. University
Press, Princeton, NJ.
Browder, F. (1961): On the spectral theory of elliptic differential operators. Math. Ann.
142, 22 130.
Browder. F. (1962): /:unctional anal)'sis and differential equations. Math. Ann. 138,
55 -19; 145, 81 - 226.
Browder, F. (1963): Nonlinear elliptic boundar}' t'alue prohlems. Bull. Amer. Math. Soc.
69, 862 874.
Browder, F. (1963a): Variational boundar.\' I'alue problems for quasilinear elliptic differ-
ential equations of arhitrary order, I-III. Proc. Nat. Acad. Sci. U.S.A. SO, 31 - 37;
592 - 598; 794 - 798.
Browder, F. (1963b): 1'he soll'abilit y of nonlinear .functional equations. Duke Math. J.
30, 557 - 566.
Browder, F. (1965): ExistelJce and uniqueness theorems for solutions of nonlinear
houndarJ'-l'alut' problems. Proc. Sympos. Appl. Math. 17,24-49. Amer. Math. Soc..
Providence, R I.
Browder, F. (1966): On the unrication of the calculus of I'ariations and the theory of
monotone nonlinear operators in Banach spaces. Proc. Nat. Acad. Sci. U.S.A. 56,
419-425.
Browder, F. (1966a): Problemes non 1i1Jeaires. Les Presses de J'Universite de Montreal.
Browder, F. (1967): Appro:{imation-so/('ability of nonlinear functional equations in
normed linear spaces. Arch. Rational Mech. Anal. 26, 33-42.
Browder, F. and Petryshyn, W. (1967): Construction of fixed points of nonlinear
mappings in Hilbert space. J. Math. Anal. Appl. 20, 197 -228.
Browder, F. (1968): Cf. Browder, F. (1968/76).
Browder, F. (1968a): Nonlinear maximal monotone mappings in Banach ,"paces. Math.
Ann. 17S, 81-113.
Browder, F. (1968/76): Nonlinear Operators and Nonlinear Equations of Evolution in
Banach Spaces. Proc. Sympos. Pure Math., Vol. 18, Part 2 (the entire issue). Amer.
Math. Soc., Providence, RI, 1976 (preprint version 1968).
Browder. F. (1969): Remarks on nonlinear interpolation in Banach spaces. J. Funct. Anal.
4, 390-403.
Browder, F. (1969a): Nonlinear ('ariatio'Jal inequalitie.'t and maximal monotone mappings
in Banach sp{l('es. Math. Ann. 183, 213 - 231.
Browder, F. and Gupta, C. (1969): Afonotone operators and nonlinear integral equation...
of Hammerstein type. Bull. Amer. Math. Soc. 7S, 1347 1353.
Browder, F. and Petryshyn. W. (1969): Approximation methods and the generalized
degree for nonlinear mappings in Banach spaces. J. Funct. Anal. 3. 217 - 245.
Browder, F. (1970): E:(istence theorems .for nonlinear partial differential equations.
Proc. Sympos. Pure Math., Vol. 16, pp. 1 - 62. Amer. Math. Soc., Providence, RI.
Browder, F. (1970a): Pseudomonotone operators and the calculus of (:ariat;ons. Arch.
Rational Mech. Anal. 38, 268 277.
Browder, F. [ed.] (1970b): Functional Anal.'sis and Related Fields. Springer- Verlag"
New York.
Browder, F. [ed.] (197Oc): Functional Anal}'sis. Proc. Sympos. Pure Math., Vol. 18,
Part I. Amer. Math. Soc., Providence, RI.

1126
References
Browder, F. (1971): Nonlinear functional analysis and nonlinear integral equations of
Ham",erste;n and Urysohn type. In: Zarantonello, E. [ed.] (1971), pp. 425-500.
Browder. F. (197Ia): Normal solvability and the Fredholm alternatives for mappings into
infinite-dimensional manifolds. J. Funct. Anal. 8, 250-274.
Browder, F. and Hess, P. (1972): Nonlinear mappings of monototle type. J. Funct. Anal.
II. 251 - 294.
Browder, F. (1975): The relation (f func.tional analysis to (.oncrete analysis in 20th
century mathematics. Historia Math. 2, 577 - 590.
Browder. F. [ed.] (1976): Mathematical Developments Arising from Hilbert's Problems.
Amer. Math. Soc., New York.
Browder. F. (1977): On a principle of H. Brezis and ilS applications. J. Funet. Anal. 25,
356 365.
Browder. F. (1978): Image d'un operateur maximal monotone et Ie principle de
l..andesmatl- Lazer. C. R. Acad. Sci. Paris, Sere A 287, 715- 718.
Browder, F. (1983): Fixed-point theory atld nonlinear problems. In: Browder, F. [ed.]
(1983a), Part 2. pp. 49- 87.
Browder, F. [ed.] (1983a): The Mathematical fleritage of Henri Poincare. Proc.
Sympos. Pure Math.. Vol. 39, Parts I, 2. Amer. Math. Soc., Providence, RI.
Browder, F. (1984): Coincidence theorems, minimax theorems, and variational inequal-
ities. Contemp. Math. 26, 67 80.
Browder, F. [ed.] (1986): NOlllinear Functional Analysis and Its Applications. Proc.
Sympos. Pure Math., Vol. 45, Parts 1,2. Amer. Math. Soc., Providence, RI.
Bruck, R. (1983): Asymptotic hehal';or of nonexpansive mtlppings. Contemp. Math. 18,
I - 47.
Bullough, R. and Caudrey, P. [eds.] (1980): Solitons. Springer- Verlag, New York.
Burenkov, V. (1976): On a method o.l extellding differentiable functions. Trudy Mat.
Inst. Steklova, Akad. Nauk SSSR 140. 27 - 67 (Russian).
Burenkov, V. (1985): The extension of functions under preservation of the Sobolev
sem;norm. Trudy Mat. Inst. Steklova, Akad. Nauk SSSR 172,71-84 (Russian).
Burkhardt, H. and Meyer, F. (1900): Potentialtheorie. In: Etlcyklopadie der Mathe-
mat ischen W;ssenschaften ( 1 899fT), Vol. 2, Part 1.1. pp. 464 - 503.
Butcher, J. (1987): Tile Numerical Analysis of Ordinary Differe,uial Equations. Wiley,
New York.
Butzer, P. and Berens, H. (1967): Semigroup... of Operators atld Approximations.
Springer- Verlag, Berlin.
Carrarelli, L., Nirenberg, L.. and Spruck, J. (1984/88): The Dirichlet prohlem for non-
linear second-order elliptic equations, I.V. Comm. Pure Appl. Math. 37,369-402:
41,47 -70.
Calderon, A. and Zygmund, A. (1956): On singular integral.... Amer. J. Math. 78,
289-309.
Calogero, F. and Degasperis, A. (1982): Spectral Transform and Solitons. North.
Holland, Amsterdam.
Calude, C. ( 1984): Theories of Computational Complexit y. North-Holland, Amsterdam.
Cannon, J., Ford, W., and Lair, A. (1976): Quasilinear parabolic systems. J. Differential
Equations 20, 441-472.
Carasso, C. and Raviart. P. [eds.] (1987): Nonlinear Hyperboli(. Problems. Lecture
Notes in Mathematics Vol. 1270. Springer-Verlag, Berlin.

References
1127
Carleman, T. (1923): Sur les equations integrales ...ingulieres a noyau reel et symetrique.
U ppsala.
Caron, R. (1969): Ahstract Methods in Partial Differential Equations. Harper and Ro\\',
New York.
Caroll, R. and Showalter, R. (1976): Singular and Degenerate Cauchy Problems.
Academic Press. New York.
Carrier. G. and Pearson. C. (1976): Partial Differential Equations. Academic Press.
New York.
Cea. J. (1964): Approximation I'ariationelle des problemes aux limite.... Ann. Inst. Fourier
14, 345-444.
Cea, J. (1971): Optim;sation: theorie et algorithmes. Dunod, Paris.
Cesari, L. (1964): Functional analysis atld Galerkin's method. Michigan Math. J. II,
385 -414.
Cesarl, L. (1976): f'unctional analysis, nonlinear differential equations, and the alternatire
met hod. In: Cesari, L. [ed.], Nonlinear F uncI ional Anal ys;s, pp. I 197. Leet u re
Notes in Pure and Applied Mathematics. Vol. 19. Dekker, New York.
Cesari. l. et al. [eds.] (1978): Nonlinear Anal}'sis: A Collection of Papers in HOllor (f
Erich Rothe. Academic Press, New York.
Cesari. L. [ed.] (1987): Contributions to the Modern Calculus of Variations. Pitman,
London.
Chazarain. J. and Pirion. A. (1982): Introduction to the Theory of Linear Partial
Differential Equations. North-Holland, Amsterdam.
Cheney, E. (1967): Introduction to Appro:(imat;on Theory. McGraw-Hili. New York.
Chern. S. [ed.] (1984): Semitlar on Nonlillear Partial Differential EqucJtions. Springer-
Verlag, New York.
Choquet-Bruhat, Y. and Yorke, J. (1980): 1he CaucllY prohlem for the Einstein equa-
tions. In: Held. A. [ed.], General Relativity and Grat,itation, Vol. L pp. 99-136.
Plenum. New York.
Choquet-Bruhat. Y. and Christodoulou. D. (1981): Existence o.f global solutions of the
Yang - A/ills, Higgs, and spinor field equations in (3 + I )-dimensions. Ann. Sci. Ecole
Norm. Sup. IV, 14, 481- 500.
Choquet-Bruhat, Y. and Christodoulou, D. (1982): Existence of global solutions of the
Yang - Iills- Higgs fields in 4-dimensional Minkotsk; space. I. II. Comm. Math.
Phys.83, 171-191; 193-212.
Choquet-Bruhat, Y., DeWitt-Morette. C., and Dillard-Bleick, M. (1982a): Analysis.
lanifolds, and Physics. North-Holland, Amsterdam.
Chonn, A. et ale (1978): Product formulas and numerical algorithms. Comm. Pure Appl.
Math. 31. 205-256.
Chow, S. and Hale. J. (1982): Methods of Bifurcation Theory. Springer-Verlag, New
York.
Chow, S. and Lauterbach, R. (1988): A bifurcation theorem for critical points of
variational prohlems. Nonlinear Anal. 12. 51- 61.
Christodoulou, D. (1986): Global solutions of nonlitzear hyperbolic equations for small
initial data. Comm. Pure Appl. Math. 39, 267 282.
Christodoulou, D. and Klainerman, S. (1990): Nonlinear Hyperbolic Equations (mono-
graph to appear).
Ciarlet, P.. Schultz. M.. and Varga, R. (1969): Numerical methods of high order accuracy
for nonlinear boundary I'alue prohlems. Numer. Math. 13, 51- 77.
Ciarlet. P. (1977): Numerical Analysis of the finite Element Method for Elliptic
Boundary Value Problems. North-Holland, Amsterdam.

1128
Rererences
Ciarlet, P. (1988): Mathematical Elasticity, Vols. 1,2. North-Holland, Amsterdam.
Ciarlct, P. and Lions, J. [eds.] (1988): Handbook of Numerical Analysis. Vol. I: Finite
Elenlent Method. Vol 2: Finite Difference Method (Vols. 3fT to appear). North-
Holland, Amsterdam.
Cioranescu, I. (1974): Aplicati; de dualitate in analiza !u,rctionald nelin;ara. Editura
Academiei, Bucuresti.
Clarke, F. (1983): Optimization and Non-Smooth Analys;s. Wiley, New York.
Clarkson, J. (1936): Uniformly convex spaces. Trans. Amer. Math. Soc. 40, 396-414.
Clement, P. el al. (1987): One-Parameter Sem;groups. North-Holland, Amsterdam.
Coddington, E. and Levinson, N. (1955): Theory of Ordinary Differential Equations.
McGraw-Hili, New York.
Cohen, P. and Feigenbaum, E. (1981): Handbook of Arti/iciallntellige,rce, Vols. 1 - 3.
Addison- Wesley, New York.
Coleman, T. (1984): lArge Sparse Numerical Optimization. Lecture Notes in Computer
Science, Vol. 165. Springer-Verlag, New York.
Colombeau, J. (1984): New Generalized Functions and Multiplication of Distributions.
North-Holland, Amsterdam.
Colombeau, J. (1985): Elementary Introdu('tion to New Generalized Functions. North-
Holland, Amsterdam.
Collatz, L. (1960): The Nllmerical Treatment of Ordinary Differential Equations.
Springer- Verlag, Berlin.
CoUatz, L. (1963): Eigenwertaufgaben mil technischen A'iwendungen. Geest & Portig,
Leipzig.
Colla tz, L. (1964): F unkt ionalanal ysis und numerische Mat hemat ik. Spri nger- Verlag.
Berlin.
Connes, A. (1977): Sur la theorie non-commutative de I'integration. Lecture Notes in
Mathematics, Vol. 725. Springer- Verlag, Berlin.
Courant, R. (1920): Ober die Eigellwerte be; den Differentialgleichungen der mathe-
matischen Physik. Math. Z. 7, I-57.
Courant, R., I-riedrichs, K., and Lewy, H. (1928): 0 ber die partiellen Differential-
gleichungen der mathematischen Physik. Math. Ann. 100,32- 74.
Courant, R. and Hilbert, D. (1937): Methoden der mathematischen Physik, Vol. 2.
Springer- Verlag, Berlin.
Courant, R. (1943): Variatio1tal nlethod... for the solution of problems of equilibriunl and
l'ibrations. Bull. Amer. Math. Soc. 49, 1 - 23.
Courant, R. (1950): Dirichlet's Principle, Conformal AI appings, and Minimal Surfaces.
In terscience, New York.
Courant, R. and Hilbert, D. (1953): Methods of Mathematical Physics, Vols. 1, 2.
Interscience, New York.
Crandall, M. and Pazy, A. ( 1969): Semigroups of nonlinear contractions and dissipative
set.. Israel J. Math. 21,261-278.
Crandall, M. and liggen, T. (1971): Generation of semigroups of nonlinear transfor-
mations on general Banach spaces. Amer. J. Math. 93, 265- 298.
Crandall, M. and Evans, L. (1975): On the relation of the operator 0/(1s + (1/t't to
el'olution governed by accretive operators. Israel J. Math. 21, 261- 278.
Crandall, M. [ed.] (1978): No,dinear Et'olution Equations. Academic Press, New York.
Crandall, M. and Lions, P. (1983): Viscosity solutions of Ilamiiton-Jacohi equatio,rs.
Trans. Amer. Math. Soc. 277, I -42.

References
1129
Crandall, M. and Lions, P. (1985): Hamilton-Jacobi equations in infinite dimensions,
1- III. J. Funct. Anal. 63, 379 - 396; 65, 368 -405.
Crandall, M. (1986): Nonlinear sem;groups and el,olution governed b}' accretive opera-
tors.ln: Browder, fo.. [ed.] (1986), Part I. pp. 305-338.
Crandall, M. and Sougandis, P. (1986): Convergence of differe'lce approximations uf
quasilinear et'olution equations. Nonlinear Anal. 10, 425-445.
Crandall, M., Rabinowit P., and Turner. R. [eds.] (1987): Directions in Partial
Differential Equations. Academic Press, New York.
Cronin. J. (1973): Equation,,, ,,'ith bounded nonlinearities. J. Differential Equations 14,
581 - 596.
Crouch, S. and Storfield. A. (1983): Boundary Element Methods in Solid Mechanics.
Allen, London.
Cryer, C. (1982): Numerical FInctional A'Jalysis. Clarendon, Oxford.
Dacarogna, B. (1982): Weak r'ontinuity and Weak Lo"'er Se,nicontinuity of Nonlinear
Funclionals. Lecture Notes in Mathematics. Vol. 922. Springer- Verlag, Berlin.
Dafermos, C. and Hrusa. W. (1985): Energy methods for quasilinear hyperholic initial-
l'ulue prohlelns: Applications to elastodynamics. Arch. Rational Mech. Anal. 87,
267-292.
Dafcrmos, C. (1986): Quasilinear systems with i1u'olutions. Arch. Rational Mech. Anal.
94. 373- 389.
Dancer, E. and Hess. P. (1988): On stable solut ions uf quasilinear periodic - paraholic
problems (to appear).
Dautray, D. and Lions, J. (1984): Analyse mathematique et falcul numerique pour les
sciences et les techniques, V ols. 1 3. Masson, Paris.
Davies. E. (1980): One-Parameter Semigroups. Academic Press, New York.
Davis, P. and Hersh. R. (1981): The Mathematical Experience. Birkhauser, 80ston, MA.
Davis, P. and Hersh, R. (1987): Descartes' Dream: The World Accordi"g to Mathematics.
Houghton Minin Company, Boston, MA.
Davydov, A. (1984): Solitons in Molecular Systems. Reidel, Boston, MA.
Debrunner, H. and Flor, P. (1964): Ein ErK'eiterungssatz fUr monotone Menge,,, Arch.
Math. 15,445 - 447.
De Figueiredo, D. (1971): An existence theorem for pseudomonotone operator equations.
J. Math. Anal. Appl. 34. 151-156.
De f"igueiredo, D. and Karlovitz, L. (1972): The extension of contractions and the
intersection 0.( balls in Banach spaces. J. Funct. Anal. 11. 168-178.
De Figueiredo, D., Lions, P., and Nussbaum, R. (1982): A priori estimates and existence
of positive solutions of semilinear elliptic equations. J. Math. Pures Appl. 61,41-63.
De Figueiredo, D. and Solimini, S. (1984): A ('ariational approach to superlinear elliptic
problems. Comm. Partial Differential Equations 9, 699- 717.
De Figueiredo. D. (1988): Lectures on the Ekeland Variational Principle with Applica-
tions and Detours. International Centre for Theoretical Physics, Trieste, Italy.
De Giorgi, E. (1957): Sulla differenziahilitd e I'analit;cita delle estremali degli integrali
multipli regolari. Mem. Accad. Sci. Torino 3, 25-43.
De Giorgi, E. ( 1968): Un esempio di estremali discontinueperunproblemal.ariazionale
di tipo ellittico. Boll. Un. Mat. Ital. (4) 1,135 137.
De Giorgi. E. (1983): G-operators and f-convergence. In: Warsaw (1983), Vol. 2.
pp. 1175 1191.

1130
References
Deimling, K. (1985): Nonlinear Functional Analysis. Springer- Verlag, New York.
Dekker, K. and Verwer, J. (1984): Stability of Runge - K utta Methods for Stiff Nonlinear
Differential Equations. North-Holland, Al1)sterdam.
Delves, L. and Walsh, J. (1974): Numer;(:al Solution of Integral Equations. Clarendon,
Oxford.
Deuel, J. and Hess, P. (1975): A criterion for the existen('e of solutions of non-linear
elliptic boundary value problems. Proc. Roy. Soc. Edinburgh Sect. A 74,49-54.
Deuel, J. (1978): Non-linear parabolic boundary-t'alue problems with sub- and super-
solutions. Israel J. Math. 29, 92 104.
Dieudonne,J.(1968): Foundatio"sof Modern Analysis, Vols. I 9. Academic Press, New
York.
Dieudonne. J. (1978): Abrege d'h;stoire des mathematiques, 1700-/900, Vols. I, 2.
I-Iermann, Paris.
Dieudonne, J. (1981): History of Functional Analysi.. North-Holland, Amsterdam.
Dinculeanu, N. (1966): Vector Measures. Akademie-Verlag, Berlin.
DiPerna, R. (1983): Convergence of the viscosity method for isentropic gas dynamics.
Comm. Math. Phys. 91, I - 30.
Ditzian, Z. and Totik, V. (1987): Moduli of Smoothlless. Springer- Verlag, New York.
Dobrowolski, M. (1980): L'L -convergence of linear finite element approximations to
nonlinear parabolic equations. SIAM J. Numer. Anal. 17, 663 - 674.
Dobrowolski, M. and Rannacher, R. (1980): Finite element methods for nonlinear
elliptic systems of se('olld order. Math Nachr. 94, 155 172.
Dodd, R. et al. (1982): Solitons and Nonlinear Waves. Academic Press, New York.
Dolezal, V. (1979): Monotone Operators and Appli('ations in ("ontrol and Network
Theory. Elsevier, New York.
Douglas, J. and Dupont, T. (1970): Galerkin methods for parabolic equations. SIAM
J. Numer. Anal. 7, 575-626.
Dubinskii, Ju. (1968): Quasilinear elliptic and parabolic equations of arbitrary order.
Uspekhi Mat. Nauk 23 (139),45- 90 (Russian).
Dubinskii, Ju. (1976): Nonlinear elliptic and parabolic equations.ltogi Nauki i Tekhniki,
Sovremennye problemy matematikii 9, 1-130 (Russian).
Dubinskii, J u. (1984): Sobolev Spaces of I nfin;te Order and Differential Equations.
Teubner, Leipzig.
Dubrovin, D., Matveev, V., and Novikov, S. (1976): On nonlinear equations of the
Korteweg de Vries type. Uspekhi Mat. Nauk 31 (I), 55-136 (Russian).
Duff, I., Erisman, A., and Reid, J. (1986): Direct Methods for Sparse Matrices.
Clarendon, Oxford.
Dunford, N. and Schwart J. (1958): Linear Operators, V ols. I 3. I nterscience, New
York.
Eardley, D. and Moncrieff, V. (1982): The global existence of Yang- Mills-lliggs fields
;n 4-dimensional Minkowski space. Comm. Math. Phys. 83,171-191; 193-212.
Eberlein, W. (1947): Weak compactness in Bana£'h spaces. Proc. Nat. Acad. Sci. U.S.A.
33, 51 - 53.
Edwards, R. (1965): FU,lctiol1al Analysi.... Holt, Rinehart, and Winston, New York.
Ehrenpreis, L. (1954): Solutions of some problems of division. Amer. J. Math. 76.
883-903.
Ehrling, G. (1954): On a type of eigenvalue problems for certain differential operators.
Math. Scand. 2,267-287.

References
1131
Eichhorn, J. (1988): Elliptic operators on non-compact manifolds. In: Schulze, B. and
Triebel, H. [eds.], Seminar Anal}'sis 1986/87. pp. 4- J 70. Teubner, Leipzig, 1988.
Ekeland. I. and Temam. R. (1974): Anal.vse convexe et problemes variationnels. Dunod,
Paris. (English edition: North-Holland, New York, 1976.)
Enc.vklopiidie der M athematischen Wissenschaften (I 899fT): Edited by A. Burkhardt,
W. Wirtinger, and R. Fricke. Teubner, Leipzig.
Ericksen, J. et al. [eds.] (1986): Homogenization and Effectit,e Moduli of Materials.
Springer- Verlag, New York.
Ericksen, J. and Kinderlehrer. D. [eds.] (1987): Theory and Applications of Liquid
Cr.'stals. Springer- Verlag, New York.
Escobar. J. (1987): Positil'e solution..fi for some semilinear elliptic equations with critical
Sobo/el' exponents. Comm. Pure Appl. Math. 40. 623-657.
Euler, L. (1744): Methodus inven;endi lineas curt'as maxim; m;n;m;re proprietate gau-
dentes, sive .fiolution problematis ;.fioperimetrici latissimo sensu accept;. Lausanne.
(German Translation: See SHickel, P. (1894)).
Euler, L. (1911): Opera omnia (Collected Works), Vols. )-72. Leipzig-Berlin; later
Basel- Zurich.
Evans, L. (1982): Classical solutions of fully nonlinear, convex, second-order elliptic
equations. Comm. Pure Appl. Math. 35,333-363.
Evans. L. (1983): Classical solutions of the Hamilton-Jacobi- Bellman equatio,I.'t for
uniformly elliptic operator.fi. Trans. Amer. Math. Soc. 121, 211- 232.
Faddeev, L. and Takhtadjan. L. (1987): The Hamiltonian Approach to the Theory of
Solitons. Springer- Verlag, New York.
Fattorini, H. (1983): The Cauchy Problem. Addison- Wesley, London.
Federer. H. (1969): Geometric Measure Theory. Springer-Verlag, New York.
f"eistauer. M., Mandel, J., and Necas, J. (1985): Entropy regularization of the transonic
flow problem. Comment Math. Univ. Carolin. 25 (3), 431-443.
Feistauer, M. (1987): On the finite element approximatio'l of a cascade floK. problem.
Numer. Math. 50,655-684.
Feistauer, M. and 2eniek. A. (1988): Compactness method in the finite element theory
of nonlinear elliptic problems. Numer. Math. 52, 147-163.
Feistauer, M. and Netas. J. (1988): Viscosit}' method in transonic f70M,'. Comm. Partial
Differential Equations (to appear).
Feng Kang (1983): Finite element method and natural houndary reduftion. In: Warsaw
(1983 ), Vol. 2, pp. 1439-1453.
Fenyo, S. and Stolle, H. (1982): Theorie und Praxis der linearen Inlegralgleichungen,
V ols. I - 4. Veri. der W iss., Berlin.
Fichera, G. (1965): Linear Elliptic Differential Systems and Eigenvalue Prohlems.
Lecture Notes in Mathematics, Vol. 8. Springer- Verlag, Berlin.
Fichera, G. (1978): Numerical and Quantitatit'e Analysis. Pitman, London.
....ichtenholl--. G. (1972): Differential- und Integralrechnung, Vols. 1-3. VerI. der Wiss.,
Berlin. (Modified English edition: Cr. Fikhtengol'ts, G. (1965).)
Fife. P. (1979): Mathematical Aspects of Reacting and Diffusing Systems. Lecture Notes
in Biomathematics, Vol. 28. Springer-Verlag, Berlin.
Fikhtengol'ts. G. (1965): The Fundamentals of Mathematical Ana/}'sis, Vols. I, 2.
Pergamon, Oxford.
Finn, R. (1985): Equilibrium Capillar)' Surfaces. Springer- Verlag, New York.

1132
References
Fischer, E. (1905): Ober quadratische f.ormen mit reel/en Koeffizienten. Monatsh. Math.
Phys. 16, 234- 249.
Fischer, A. and Marsden, J. (1972): The Einstein evolution equations as a first-order
quasilinear symmetric hyperbolic system. Comm. Math. Phys. 28, 1-38.
Fitzpatrick, P., Hess, P., and Kato, T. (1972): Local bou'ldedness oj' nlonotone-t ype
operators. Proc. Japan Acad. 48, 275- 277.
Fitzpatrick, P. (1973): Surjectit,ity results from a Banach space to its dual, Math. Ann.
204, I 77 - 188.
F'itzpatrick, P. and Petryshyn, W. (1975): f.;xed-point theorenls and the fixed-poilu
index for multit'alued nlapp;'lgs in cones. J. London Math. Soc. 12, 75 -85.
Fitzpatrick, P. (t 977): ('ontinuity of nonlinear monotone operators. Proc. Amer. Math.
Soc. 62, III -116.
Fitzpatrick, P. (1978): Existence results for equations involving noncompact perturba-
tions of Fredholm mappings. J. Math. Anal. Appl. 66, 151 -177.
J.'itzpatrick, P. and Petryshyn, W. (1978): 0,1 the nonlinear eigenvalue problem Tu = ACU
involving abstract and differential operators. Boll. Un. Mat. Ital. 158. 80-107.
Fitzpatrick, P. and Petryshyn, W. (1979): Sonle applications oj" A-proper mappings.
Nonlinear Anal. J. 525 - 532.
Fitzpatrick, P. and Petryshyn, W. (1982): On connected compone,us of solutions of
Ilonlinear eigenvalue problenl..'i. Houston J. Math. 8, 477 - 500.
Fleming, W. (1986): Controlled Marko(' Processes and Viscosity Sulutions of Nonlinear
Evolution Equations. Lezione Fermiane, Pisa.
Fletcher, C. (1984): Computational Galerkin Methods. Springer- Verlag, New York.
f.lorian, H., Perktold, K., and Gruber, K. (1984): Sinlulation der Partikelbahnen inl
pulsierenden Blutstrom einer Carotis- Verzweigung und finite Elemente. Sitzungs-
her. Osterr. Akad. Wiss. Math.-Naturwiss. KI., Vol. 193, pp. 105-113.
Forsythe, G. and Wasow, W. (1960): Finite Difference Methods for Partial Differential
Equations. Wiley, New York.
Fortin, M. and Glowinski, R. [eds.] (1983): Augnlented Lagrangian Methods: Appli-
cation to the Numerical Solution of Boundary- Value Problems. North-Holland,
Amsterdam.
Fourier. J. (1822): La theorie analytique de la chaleur. Didot, Paris.
.rank, P. and von Mises, R. (1961): Die Differential- und Integralgleichungen der
Mechanik und Physik. Vieweg, Braunschweig.
"redholm.l. (1900): Sur une nouvelle methode pour la resolutioll du probleme"de Dirichlet.
Kong. Vetenskaps-Akademiens Forh. Stockholm, pp. 39-46. .
"redholm, I. (1903): Sur une class d'equations fonctionelles. Acta Math. 27, 365 390.
Freed, D. and Uhlenbeck, D. (1984): Instantons and Four-Manifolds. Springer-Verlag.
New York.
F'rehse, J. ( 1977): Opt imale gleichmiiftige K onvergenz der Methode der fin; t en Elenle,u e
be; quas;/inearen n-dimensionalen Randwertproblemen. Z. Angew. Math. Mech. 57,
229 - 23 1.
f-.rehse, J. (1977a): On Signorini's problem and variational problems with thin obstacles.
Ann. Scuola Norm. Sup. Pisa CI. Sci., Sere IV, 4, 343-362.
Frehse, J. and Rannacher, R. (1978): Asymptotic Loo -error estimates for linear finite
elenlent approxinlations of quasilinear boundary-value problems. SIAM J. Numer.
Anal. 15,418-431.
Frehse, J. (1982): Zur asymptotischen Konvergenz der Methode der f;niten Elemenle.
Mitt. Math. Ges. DDR, 1982, Heft 3/4, pp. 8-30.

References
1133
Frehse, J. (1982a): Capacity methods i" the theory of partial differential eql4ations.
Jahresber. Deutsch. Math.- Verein. 84, I 44.
Frehse, J. (1983): Uniform asymptotic error estimates for finite element approximations.
In: Proceedings of the China - France Symposium on Finite Elements. Science
Press, Beijing, China, pp. 253 - 264.
Ftehse, J. (1983a): On the smooth,Jes.4i of solutions of variationlll inequalities ,;th
obstacles. Banach Center Publications, Vol. 10, PWN, Warsaw, pp. 87 128.
Friedlander, F. (1976): The ",'ave Equation on a Curved Space - Time. University Press,
Cambridge, England.
Friedman, A. (1964): Partial Differential Equations o.{ Parabolic Type. Prentice-Hall,
Englewood Cliffs, NJ.
Friedman, A (1969): Partial Differential Equations. Holt, Rinehart. and Winston, New
York. (Second edition 1976.)
Friedman, A. (1982): Variational Principles a'ld Free-Boundary- Value Problems. Wiley,
New York.
Friedrichs, K. (1929): Ein Jler{ahren der Variationsrechnung das Minimum eines Integrals
als das A-faxinJum eines anderen Ausdrucks darzustellen. Nachr. Ges. Wiss.
Gottingen, Math.-Phys. KI., pp. 13 20.
J."ricdrichs, K. (1934): Spektraltheorie halbbeschrankter Operatoren, I. II. Math. Ann.
109, 465-487; 685 - 713.
Friedrichs, K. (1939): On differential operators in Hilbert splIces. Amer. J Math. 61,
523-544.
Friedrichs, K. (1944): The identit)' of y.'eak and strong extensiolls of differential upera-
tors. Trans. Amer. Math. Soc. 55, 132 - 153.
Friedrichs, K. (1953): On the differentiability of solutions of linear elliptic differential
equations. Comm. Pure Appl. Math. 6,299-326.
Friedrichs, K. (1954): Symmetric hyperbolic linear differential equatio'is. Comm. Pure
Appl. Math. 7, 345 - 392.
Friedrichs, K. (1980): on Neumann's Hilbert space theory and partial differential
equations. SIAM Rev. 22,486-493.
Friedrichs, K. (1986): Selecta, V ols. I, 2 (Selected Works). Birkhauser, Boston.
Fucik, 5., Necas, J., SouCek, J. & V. (1973): Spectral Analysis of Nonlinear Operators.
Lecture Notes in Mathematics, Vol. 346. Springer- Verlag, Berlin.
Fucik, 5., Kratochvil, A., and Necas, J. (1973): The Kacanov-Galerkin method.
Comment. Math. Univ. Carolin. 14,651 659.
F'ucik, S. (1977): Ranges of Nonlinear Operators. Lecture Notes, Prague University.
See Fucik, S. (1981).
Fucik, S. and Kufner, A. (1980): Nonlinear Differential Equations. Elsevier, New York.
Fucik, S. (1981): Soll'abilit}' of Nonlinear Equations and Boundary Value Problen.s.
Reidel, Boston.
Fujita, H. and Kato, T. (1964): On the Nat 1 ier-Stokes initial-value problem. Arch.
Rational Mech. Anal. 16. 269- 315.
Funk, P. (1962): Variationsrechnung und ihre Anwendung in Physik und TechtJik.
Springer- Verlag, Berlin.
Gagliardo, E. (1959): Ulterior; proprieta di alrune classi di .[unzioni in piu l'ariabli.
Ricerche Mat. 8, 102 - 137.
Gaines, R. and Mawhin, J. (1977): Coincidence Degree and Nonlinear Differential
Equations. Lecture Notes in Mathematics, Vol. 568. Springer-Verlag, Berlin.

1134
References
Gajewski, H. (1970): Iterations- Projektions- und Projektions-Iterationsverfahren zur
Berechllung visco-plastischer Stromunyen. Z. Angew. Math. Mech. , 485-490.
Gajewski, H. (1970a): Ober einige Fehlerabschiitzungen bei Gleichungen mil monotonen
Potentialoperatoren in Banachriiume,1. Monatsber. Ot. Akad. Wiss. Berlin 12,
57J -579.
Gajewski, H. and Kluge, R. (1970): Projektions-Iterationsverfahren und nichtlineare
Probleme mit ,nollotorJetJ Operatoren. Monatsber. Dt. Akad. Wiss. Berlin 12,
90- 115.
Gajewski, H. (1972): Zur funktionalanalytischen Formulierung stationiirer Kriech-
prozesse. Z. Angew. Math. Mech. 52, 485-490.
Gajewski, H., Grager, K., and Zacharias, K. (1974): Nichtlineare Operatorgleichungen
und Operatordifferentialgleichungen. Akademie- Verlag, Berlin.
Gajewski, H. and Groger, K. (1986): On the basic equations for carrier transport in
selniconductors. J. Math. Anal. Appl. 113, 12- 35.
Galerkin, B. (1915): Rods and plates. Vestnik Inzenerov 19 (Russian).
Garabedian, P. (1964): Partial Differential Equations. Wiley, New Yark.
Garabedian, P. (1972/77), (1978), (1984) (1986): Cf. Bauer, F. et al. (1972/77), (1978),
(1984), (1986).
Garding, L. (1953): Dirichlet's problem for linear elliptic differential equations. Math.
Scand. 1,55- 72.
Gardner, C., Green, J., Kruskal, M., and Miura, R. (1967): Method for solving the
Korteweg-de Vries equation. Phys. Lett. 19, 1095 1097.
Gauss, C. (1839): Allgemeine Lehrsiitze uber die im verkehrten Verhaltnisse des Quadrats
der Entfernung wirkenderJ Anziehungs- und Abstoftungskriifie. In: Gauss, C. (1863),
Vol. 5, pp. 195 - 242.
Gauss, C. ( 1863): Werke (C o/lected Works), V ols. I - 12. Gottingen, 1863-1929.
Gear, C. (1971): N umericallnitial- Value Problems in Ordinary Differential Equations.
Prentice-Hall, Englewood Cliffs, NJ.
Gekeler, E. (1984): Discretization Methods for Stable Initial- Value Problen.s. Lecture
Notes in Mathematics, Vol. 1044. Springer- Verlag, Berlin.
Gelfand, I. and Silov, G. ( 1964): Generalized F unct ions, V ols. I - 5. Academ ic Press, New
York.
Germain, P. and Nayroles, 8. [eds.] (1976): Applications of Methods of Functional
Analysis to Problems in Mechanics. Lecture Notes in Mathematics, Vol. 503.
Springer- Verlag, Berlin.
Giaquinta, M. (1983): Multiple Integrals in the Calculus of Variations and Nonlinear
Systems. University Press, Princeton, NJ.
Giaquinta, M. and Hildebrandt, S. (1989): The Calculus of Variations, Vols. 1- 3
(monograph to appear).
Gidas. B., Ni, W., and Nirenberg, L. (1979): Symmetry and related properties via the
nJaximum principle. Comm. Math. Phys. 68,209--243.
Gilbarg, D. and Trudinger, N. (1983): Elliptic Partial Differential Equations of Secolld
Order. Springer-Verlag, New York (second enlarged edition).
(jinibre, J. and Velo, G. (1983): 011 the global Cauch.v problem for some nonlinear
SclJrodinger equations. Ann. Inst. H. Poincare. Anal. Non Lineaire I, 309 - 324.
Ginibre, J. and Velo, G. (1986): The Cauchy problem in the energy space for the 'lo,J/inear
SclJrodinger and Klein - Gordon equat ions. In: Brezis, H., Crandall, M., and Kappel,
F. [cds.] ( 1986), Vol. I, pp. 121- 133.
Girault, V. and Raviart, P. (1986): Finite Element Approxirnation of the Nat'ier-Stokes
Equat ions. Springer-Verlag, New York.

References
1135
Gittel, H. (1987): Studies on transonic ./10....' problems h}' nonlinear variational inequalities.
Z. Anal. Anwendungen 6,449-458.
Giusti, E. and M irand C. (1968): Sulla regularitti delle soluzioPli deholi di una classe
di sistem; ellittici quasilineari. Arch. Rational Mech. Anal. 31, 173-184.
Giusti, E. (1984): Minimal Surfaces and f"unctions of Bounded Variation. Birkhauscr,
Boston.
Giusti, E. [ed.] (1985): Harmonic Afappi'lgs and Minimal Immersions. Lecture Notes in
Mathematics, Vol. 1161. Springer-Verlag, Berlin.
GlashofT, K. and Werner, B. (1979): Inverse monotonicit}' of monoto'Je L-operators with
applications to quasilinear and free boundar.' value problems. J. Math. Anal. Appl.
72,89-105.
Glassey, R. and Strauss, W. (1987): Ab.ence of shocks in an initially dilute coUis;onless
plasma. Comm. Math. Phys. 113, 191-208.
Glimm, J. (1965): Solutions in the large for nonlinear hyperbolic systems of equations.
Comm. Pure Appl. Math. 18, 697 - 715.
Glimm, J. and Jaffe, A. (1981): Quantum Physics. Springer-Verlag, New York.
Glimm, J. (1988): The interaction 0.( nonlinear hyperbolic waves: a survey. Comm. Pure
Appl. Math. 41, 569-589.
Glowinski, R. and Lions. J. [eds.] (1974): Computing A-lelhods in Applied Sciences and
Engineeri'lg, I -VII. Springer-Verlag, New York, 1974, 1976,1977,1979; North-
Holland, Amsterdam, 1980, 1986.
Glowinski, R., Lions, J., and Tremolieres, R. (1976): Analyse numerique des inequations
l'ariat;onelles, V ols. I. 2. Gauthier. V illars. Paris. (English edition: North-Holland,
Amsterdam, 1981.)
Glowinski, R. (1984): Numerical Methods for Nonlinear Variational Problems. Springer-
Verlag, Ne\\' York.
Godunov, S. and Ryabenkii, V. (1987): Difference Schemes. North-Holland, Amsterdam.
Golomb, M. (1935): Zur Theorie der nichtlinearen InlegralgleiclJungen. Math. Z. 39,
45- 75.
Gould, S. (1966): Variational Methods for Eigenvalue Problems. University Press,
Toronto.
Greenberg, W. et al. (1986): Boundary Value Problems in Abstract Kinetic Theor)'.
Birkhauser, Boston.
GrigoriefT. R. (1973): Zur Theorie approximations-regularer Operatoren, I, II. Math.
Nachr. 55, 233 - 249; 251 - 263.
Grillakis, M. (1988): Linearized instabilit), .for nonlinear Schrodinger and K le;n - Gordon
equations. Comm. Pure Appl. Math. 41,747-774.
Grisvard, P. (1985): Elliptic Problems in N onsmooth Domains. Pitman, London.
Groger, K. (1986): On the existence of steady stales of certain reaction diffusion system..
Arch. Rational Mech. Anal. 92, 297 - 306.
Grager, K. (1989): Wi -Estimates for solutions to mixed boundary problems for second-
order elliptic diffe, ential equations. Math. Ann. (to appear).
Gunter, N. (1957): Die Potentialtheorie und ihre Anwendung auf Grundaufgaben der
mathematischen Physik. Teubner, Leipzig.
Gunther, P. et al. (1972): Grundkurs Analysis, V ols. 1-4. Teubner, Leipzig.
Gunther, P. (1988): Huygens' Principle for Linear Partial Differential Operators of
Second Order. Academic Press, New York.
Gunther, M. (1987): On nonlinear interpolation theory and global solutions of the
Korte....'eg- de Vries equation (unpublished paper).

1136
References
Gunther, M. (1988): Beitriige zlIr lokalen Losbarkeit partieller DifJerentialgleichungen
auf M atJtJigfaIt igkeiten. Dissertation B, Karl- Marx- U niversitat, Leipzig.
Gunther, M. (1988a): () ber eine spezielle K lasse nichtlinearer, lokal stetig invertierbarer
Operatoren. Math Nachr. 136, 167-184.
Gupta, C. (1983): SOlne theorems OIl the sum of 1J0niinear mappings of monotone type in
Banach spaces. J. Math. Anal. Appl. 97, 38-45.
Guseinov, A. and Myhtarov, H. (1980): IIltroduction to the 1'heoryof Nonlinear Integral
Equations. Nauka, Moscow (Russian).
Gustafson, K. and Sather, D. (1972): IJarge nonlinearities and monotonicity. Arch.
Rational Mech. Anal. 48, 109-122.
Hackbusch, W. (1979): On the fast solution of nonlinear elliptic equations. Numcr. Math.
32, 83 95.
Iackbusch, W. and Trottenberg, U. [eds.] (1982): Mult;grid Methods. Lecture Notes
in Mathematics, Vol. 960. Springer- Verlag, Berlin.
Hackbusch, W. (1985): Multigrid Methods and Applications. Springer-Verlag, New
York.
Hackbusch, W. (1986): Theorie und Nu"zerik elliplischer Differentialyleichungen.
Teubner, Stuttgart.
Hackbusch, W. and Trottenberg, U. [cds.] (1986): lultigrid Methods II. Lecture Notes
in Mathematics, Vol. 1228. Springer- Verlag, Berlin.
Hageman, L. and Young, D. (1981): Applied Iterative Methods. Academic Press, New
York.
Hahn, H. (1927): () ber lineare Gleichungssyste,ne in linearen Riiumen. J. Reine Angcw.
Math. 157, 214-229.
Hale, J. (1988): A.ymptotic Behavior of Dissipative Systems. Amer. Math. Soc.,
Providence, RI.
Halmos, P. (1954): Measure Theory. Van Nostrand, Toronto.
Hamilton, R. (1982): The inverse function theorem of Nash and Moser. Bull. Amer.
Math. Soc. (N.S.) 7,65-222.
Hamilton, R. (1982a): Three-manifolds with positive Ricci curvature. J. Differential
Geometry 17,255-306.
Hammerstein, A. (1930): Nichtlineare Inteyralgleichungen nebst Allwendungen. Acta
Math. 54, 117 - 176.
Haraux, A. (1981): Nonlinear Evolution Equations: Global Behavior of Solutions.
Lecture Notes in Mathematics, Vol. 841. Springer-Verlag, Berlin.
Hartman, P. and Stampacchia, G. (1966): On some nonlinear elliptic differential equa-
tions. Acta Math. liS, 271- 310.
Hawking, S. and Ellis, G. (1973): 1'lIe Large Scale Structure of Space- Time. University
Press, Cambridge, England.
Hawkins, T. (1970): History of Lebesgue's 1.heory of Integration. Chelsea, New York.
Heinrich, B. (1987): Finite Difference Methods in Irregular Networks. Akademie-Verlag,
Berlin. .
.Jeinz, E. (1986): 0 ber die Regularitiil schwacher Losungen nichtlinearer elliptischer
Systeme. Nachr. Akad. Wiss. Gottingen, Math.-Phys. KI., pp. 1 -15.
Helly, E. (1921): () ber S ysteme linearer Gleichungen mit unendlich vielen U nbekannten.
Monatsh. Math. Phys. 31,60-91.
Henry, D. (1981): Geometric Theory of Semilinear Parabolic Equations. Lecture Notes
in Mathematics, Vol. 840. Springer-Verlag, Berlin.

References
1137
Hess, P. (1971): On nonlinear equations 0.( Hammerstein type in Banach spaces Proc.
Amer. Math. Soc. JO, 308-312.
Hess, P. (1972): On the Fredholm alter'latil.'e for nonlinear fUllctional equations in Banach
spaces. Proc. Amer. Math. Soc. 33, 55-61.
Hess, P. (1973): On nonlinear problems of monotone tJ'pe K'ith respect to t'o Banach
spaces. J. Math. Pures Appl. 52. 13- 26.
Hess, P. (J 974): 0'1 a theorem b' Landesman and Lazer. Indiana Univ. Math. J. 23,
827-829.
Hess, P. (1974a): On semicoercive nonlinear problems. Indiana Univ. Math. J. 23,
645-654.
Hess, P. (1978): Nonlinear elliptic problems in unbounded domains. In: Kluge, R. [ed.]
(1978), Theory of Nonlinear Operators, C onstructit'£' Aspects, pp. 105 - 110.
Akademie- Verlag. Berlin.
Hess. P. (1981): On multiple solutions of nonlinear elliptic eigenvalue problems. Comm.
Partial Differential Equations 6, 951-961.
Iestenes. M. (1951): Applications of the theory of quadratic fornJs in Hilbert space to
t he calculus of variat ions. Pacific J. Math. I, 525 - 581.
He\\'itt, E. and Stromberg, K. (1965): Real and Abstract Anal}'sis. Springer- Verlag,
Berlin.
Hilbert, D. (1897): Die 7"heorie der alyebraischen Zahlkorper. In: Hilbert. D. (1932),
Vol. I. pp. 63 -482.
Hilbert. D. (1900): Mathematische Prohleme (Paris Lecture). German version: Cf.
Aleksandrov. P. [ed.] (1971), pp. 22-80. (English translation: Cf. Hilbert, D.
( 1902).)
Hilbert, D. (1900a): Ober das Dirichletsche Prinzip. Jahresber. Deutsch. Math.-Verein.
8, 184 - 188.
Hilbert, D. (1901): Lecture on the Dirichlet principle. Published in Hilbert. D. (1904).
Hilbert, D. (1902). Mathe,natical ProblenJs. lecture delivered before the First Inter-
national Congress of Mathematicians at Paris 1900. Bull. Amer. Math. Soc. 8.
437-479. (Reprinted in Browder, F. [ed.] (1976), Vol. I, pp. 1- 34.)
Hilbert. D. (1904): Ober das Oirichletsche Prinz;p. Math. Ann. 59.161-186.
Hilbert. D. (l904a): Grundzuge einer allyemeinen Theorie der linearen Integralglei-
chungen. Erste Mitteilung (first note). Gattinger Nachr., 1904. pp. 49-91.
(Reprinted in Hilbert, D. (1912).)
Hilbert, D. (1906): Zur Varialionsrechnung. Math. Ann. 62, 351-370.
.Iilbert, D. (1906a): Cf. Hilbert, D. (1912), Vierte und jUnfte Mitteilung (fourth and fifth
"ote).
Hilbert, D. (1910): Cf. Hilbert, D. (1912), Sechste Mitteilung (sixth note).
Hilbert, D. (1912): Grundzuge einer allgemeinen Theorie der linearen Integra/glei-
(Ohungen. Teubner, Leipzig.
Hilbert, D. (1932): Gesamme/te Abhandlungen (Collected Works). Vols. I 3. Springer-
Verlag, Berlin. (Second edition 1970.)
Hildebrandt. So (1969): Boundary behat'ior of minimal surfaces. Arch. Rational Meeh.
Anal. 35,47-82.
Hildebrandt, S. and Widman. K. (1977): On the Hiilder continuity 0.( 'eak solutions of
quasilinear elliptic systelns 0.1' second order. Ann. Scoula Norm. Sup. Pisa CI. Sci..
Ser. IV. 4,144-178.
Hildebrandt. S. (1982): LiouI,ille theorems for harmonic mappings and an approach
to the Bern.tein theorem. Annals of Mathematical Studies, Vol. 102, pp. 107 -132,
Princeton, NJ.

1 t 38
References
Hildebrandt, S. (1984): Minimal surfaces with free boundaries and related problem6-"
Asterisq ue 118, 69 88.
IIildebrandt, S. (1984a): Calculus of variatio1ls today, reflected in the Oberwolfach
nJeet i1lgs. I n: Jager, W. et al. ( 1984), pp. 321 - 336.
Ilildcbrandt, S. (1985): [eclures OIl har,nonic mappings of Riemallnian.n{nifolds.ln:
Giusti, E. [cd.] (1985), pp. 1-117.
Hildebrandt" S. and Tromba, A. (1985): Mathematics and Optimal F'orm. Scientific
American Library" "reeman, New York.
Hildebrandt, S. (1986): Free houndary value problems for minimal surfaces and related
questions. Comm. Pure Appl. Math. 39, Supplement Sill S 138.
Hildebrandt, S. and Giaquinta, M. (1989): The f"alculus of VaritJtions, Vols. 1-3
(monograph to appear).
Hildebrandt, S. and Leis, R. [eds.] (1989): Partial Differential Equatio1ls and Calculus
of Variations. Springer- Verlag, New York (to appear).
Hille, E. (1948): f'unftional Analysis and Semigroups. Amer. Math. Soc., New York.
Hille, E. and Phillips, R. (1957): f'u'lct;onal Anal}'sis and Semigroups. Amer. Math. Soc.,
Providence, R I.
Hirzebruch, F. and Scharlau, W. (1971): EilifUhrung in die Funktionalanalysis. Biblio-
graph. Inst., Mannheim.
Hirzebruch, F. (1974): Cf. Otte" M. [ed.] (1974).
Hofer, H. (1982): Variational and topological methods in partially ordered Hilbert spaces.
Math. Ann. 261, 493 514.
Hofer, H. (1984): A note on the topological degree at a critical point of Inountain-pa6'iS
type. Proc. Amer. Math. Soc. 90, 309-325.
Holmcs, R. (1975): Geometric Functional Analysis and Its Applications. Springer-
Verlag, New York.
Hormander, L. (1955): On the theory of general partial differential operators. Acta Math.
94, 162 - 248.
Hormander, L. (1964): Linear Partial Differential Operators. Springer- Verlag, Berlin.
Hormander, L. (1983): The A na I}'s is of Linear Partial Differential Operators, V ols. 1-4.
Springer- Verlag, New York.
Horvath, J. (1966): Topological Vector Spaces and Distributions. Addison-Wesley, New
York.
Hughes, T., Kato, T., and Marsden, J. (1977): Well-posed quasilinear second-order hyper-
bolic systems with applications to nonlinear elastodynanlics and general relativity.
Arch. Rational Mech. Anal. 63, 273-294.
Hiinlich, R. (1970): Ober einige Naherungsver.fahren zur IJosung nichtlinearer FU,lk-
tionalgleichungen mit N ebenbedingungen. Math. Nachr. 47, 171- t 82.
1M S L ( 1987): Software S yst em for General Classes of Mat hemal ical Problems. Houston,
Texas.
Isaacson, E. and Keller, H. (1966): Analysis of Numerical Methods. Wiley, New York.
Its, A. and Matveev, V. (1975): Schrodinger operators with finite-gap spectrum and
N-soliton solutions of the Korteweg -de Vries equation. Theoret. Math. Phys. 23 (1),
51 - 67 (Russian).
Ivanov, A. (1982): Quasilinear degenerate and non-uniformly ellipt ic and parabolic
second-order differential equations. Trudy Mat. Inst. Steklov., Leningrad, Vol. 160
(Russian).

References
1139
Jackson, D. (1912): On approximat;otl hy trigonometric sums and polynomials. Trans.
Amer. Math. Soc. 13, 491 - 515.
Jacobi, C. (1837): Cf. Stackel. P. [cd.] (1894).
Jager, W., Moser, J., and Remmert, R. [eds.] (1984J: Perspect;('es in h1athenlatics.
Blrkhauser, Basel.
Jameson, A. (1988): (.omplltat;onal Trallson;cs. Comm. Pure Appl. Math. 41,507 -550.
Janenko, N. (1969): Die Z,,'ischL'nschrillmelhode zur Lc)sung mehrdimensionaler Proh-
It'nlt' der mathen,at;schen Ph}'sik. l..ecture Notes in Mathematics, Vol. 91. Springer-
Verlag, Berlin.
Jeffrey. A and Taniuti, T. (1964): Nonlinear 'at't' PropafJation M.'ith App/icatiotls to
Ph)'sics a'id Magnetoh.,'drodytlamics. Academic Press, New York.
Jeffrey. A. ( 1976): Quasilinear II yperbolic S J!stetns and Wa.)es, Pitman, London.
John. F. (1955): Platle .yal'es and Spherical Means Applied to Partial Dfferential
Equations. Interscience. New York.
John, -. (1974): Formation (,. singularities in one-dinlens;onal notllinear \\'QI'e propaya-
I;on. Comm. Pure Appl. Math. 27, 377 405.
John, F. (1976): Delayed singular;ty formation i'l solutions oj nonlinear "'are equat;on..
in higller dimensions. Comm. Pure Appl. Math. 29, 649 - 681.
John, F. (1982): Partial Differential Equatiotls. Springer- Verlag, New York.
John, f-. (1983): l..o\\'er bounds .for the life span of solutions of nonlinear \\'al'e equations
in three dimensions. Comm. Pure Appl. Math. 36, I 35.
John, F. (1984): .4 'alk through partial differential equations. In: Chern, S. [cd.] (1984),
pp. 73 84.
John, -. and Klainerrnan. S. (1984): A Imo..t ylobal eistence to nonlinear "'are equations.
Comm. Pure Appl. Math. 37, 443 -455.
John, F. (1985): Collected Papers. Vols. I. 2. Birkhauser, Boston.
John, f:. (1986): I..ong time effects of 'JonlinearilY in second-order hyperbolic equat;o'is.
C"omm. Pure Appl. Math. 39, Supplement 5139-SI48.
John, F. ( 1987): E:;ste'Jce (or large times of ..trict solutions of nonlineur "tUft' equatio'Js
;n three dimension. for small ;nit;td data. Comm. Pure Appl. Math. 40.79-109
John, F. (1988): Almost global existence of elastic ,,'al'es of.(itl;te amplitude arising from
s",all initial disturhatlces. C'omm. Pure Appl. Math. 41, 615-666.
Josl. J. (1984): Ilarmon;c laps bet'een Surfaces. Lecture Notes in Mathematics, Vol.
1062. Springer- Verlag, Berlin.
Jost, J (1985): Lectures on Harmonic Alaps \\'ith Applications to Conformal Mappings
arrd Minitnal Surfaces. Lecture Notes in Mathematics, Vol. 1161. Springer- Verlag,
Berlin.
Jost. J. (1988): Nonlinear Methods in Riemann;an and Kiihler;tln Geometry. Birkhauser.
Boston, M A.
Jost, J. (1988a): Das E'(islenzprohlem fUr Minimalj1iichen. Jahresber. Deutsch. Math.-
Verein. (to appear).
Kacur, J. (1985): A/t'lhod of Rothe ;n Evolution Equat;oPls. Tcubner, Leipzig.
Kacurovskii, R. (1960): On motlotone operators and conve.t funet ionals. Uspek hi Mat.
Nauk 15 (4),213 - 215 (Russian).
Kacurovskii, R. (1970): On the Fredhol,n theory for nonlinear operators. Dokl. Akad.
Nauk SSSR 192,969-972 (Russian).

1140
References
Kadec, M. (1959): Spaces norm isomorphic to a locally uniformly (.onl'ex space. Izv. Vyss.
Ucebn. Zaved. Mat. 6 (13), 51- 57 (Russian).
Kamke, E. (1960): Da.fi Lebesgue- St;eltjes Integral. Teubner, Leipzig.
Kantorovic, L. (1948): fe'unct;onal analysis and applied mathematics. Uspekhi Mat.
Nauk 3 (6), 89- 185 (Russian).
Kantorovic, L. and Akilov, G. (1964): Funktionalanalysis in normierten Riiumen.
Akademie- Verlag, Berlin. (English edition: Pergamon Press, Oxford, 1964.)
Kanwal, R. (1983): Generalized ".un('t;ons. Academic Press, New York.
Karpman, V. (1977): Nichtlineare Wellen. Akademie- Verlag, Bertin.
Kato, T. (1966): Perturbation Theory for Linear Operators. Springer- Verlag, Berlin.
Kato, T. (1975): Quasilinear equations of evolution with applications to partial differential
equat;ons. Lecture Notes in Mathematics, Vol. 448, pp. 25- 70. Springer-Verlag,
New York.
Kato, T. (1975a): The Cauchy problem for quasilinear sytnmetric hyperbolic systems.
Arch. Rational Mech. Anal. 58, 181 205.
Kato, T. (1976): Linear and quasilinear equations of evolution of hyperbolic type. In:
Ilyperbolicity, Centro Internationale Matematico Estivo II Cicio 1976, Italy,
pp. 125 - 191.
Kato, T. (1983): On the Cauchy probletn for the generalized Korteweg--de Vries equa-
tiolls. In: Guillemin, V. [ed.] (1983), Studies ;n Applied Mathematics, VoJ. 8,
pp .93 - 128. Academic Press, New York.
Kato, T. (1984): Locally coercive nonlinear equations \v;th applications to some periodic
solut;ons. Duke Math. J. 51,923 -936.
Kato, T. and Lai, C. (1984): Nonlinear evolution equat;ons and the Euler flow. J. ....unct.
Anal. 56, 15- 28.
Kato, T. (1985): Abstract Differential Equations and Nonlinear Mixed Problems.
Lezione F ermiane, Pisa.
Kato, T. (1986): Nonlinear equations of evolution in B-spaces. In: Browder, F. [ed.]
(t 986), Part 2, pp. 9 - 24.
Kato, T. and Masuda, K. (1986): Nonlinear evolution equations and anal}'ticity I. Ann.
Inst. H. Poincare. Anal. Non Lineaire 3, 455-467.
Kawohl, B. (1985): Rearrangements and Convexity of l...evel Sets in Partial Differe,uial
Equations. Lecture Notes in Mathematics, Vol. 1150. Springer-Verlag, Berlin.
Kazdan, J. and Warner, F. (1975): Remarks on some quasilinear elliptic equations.
Comm. Pure Appl. Math. Z8, 567-597.
Kazdan, J. (1985): Prescribing the Curvature of a Riemannian Manifold. Amer. Math.
Soc., Providence, RI.
Kemnochi, N. (1974): Nonlinear operators of monotone type in reflexive Bana(.h spaces
alld nonlinear perturbations. Hiroshima Math. J. 4, 229 - 263.
Kenderov, P. (1976): The set-valued monotone mappings are almost everywhere single-
valued. Studia Math. 56. 199-- 203.
Kichcnassamy, S. (1987): Quasi-linear problems with s;ngular;ties. Manuscripta Math.
57, 281- 313.
Kindcrlehrer, D. and Stampacchia, G. (1980): An Introduction to Variationallnequal-
ities and 1"heir Application. Academic Press, New York.
Kirszbraun, M. (1934): Oher die kontraktiven und Lipsfhitz-sletigen Transformationen.
Fund. Math. 22, 77 -108.
Klainerman. S. and Majda. A. (1980): Formation of si,rgularities for wave equatiol1s
including the no"linear vihrati,rg string. Comm. Pure Appl. Math. JJ, 241- 263.

References
1141
Klainerman. S. and Majda, A. (1981): S;ngillar limits of quasi linear lIyperholic s}'stems
,,'ith large parameters and the incompressible limit of compressible fluids. Comm.
Pure Appl. Math. 34, 481 524.
Klainerman. S. (1983): LO'IY time behlll'ior 0.( solillions to nonlinear war'e equations. In:
Warsaw (1983). Vol. 2, pp. 1209 -1215.
Klainerman, S. and Ponce, G. (1983): Glohal s,nall amplitude solutions to nonlinear
erolution equations. Comm. Pure Appl. Math. 36. 133-141.
Klainerman, S. (1985): lll1iform decay estimates and the torelltz i'll'ariance of the
classical wave equation. Comm. Pure Appl. Math. 38, 321-332.
Klainerman, S. (1985a): Global existence of small amplitude solutions to nonlinear
Klein Gordon equations in four space - t i,ne dimensions. Comm. Pure Appl. Math.
38, 631 - 641.
Klainerman, S. (1987): Einstein geometr}' and hyperbolic equations. In: Crandall, M.,
Rabinowit P., and Turner, R. [eds.] (1987), pp. 113-144.
Klein, F. (1926): J10rlesungen uber die Geschichte der Mathematik des 19. Jallrhu,lderts.
Springer- Verlag. Berlin.
Klotzlcr. R. (1971): Mehrdimensionale Variationsrechnung. VerI. der Wiss., Berlin.
Kluge. R. (1979): Nichtlineare Variationsu1Jgleichungen und Extremalaufgaben. VerI. der
Wiss., Berlin.
Kluge. R. (1985): Zur Parameterhestimmllng in n;chllinearen Prohlemen. Teubner,
Leipzig.
Knauer, 8. (1971): U ntere Schranken fur die £i'lschliejJung selhstadjung;erler posit if'-
deJ.initer Operatorell. Numer. Math. 17, 166- 171.
Kneser. H. (1950): Eine direkte Ahleitung des Zornschen Lemmas aus dem Aus"'ahl-
axiom. Math. Z. 53, 110 113.
Knorrer. H. (1986): Integrable Hamillonsche Systeme und algebraisclJe Geonletrie.
Jahresber. Deutsch. Math.- Verein. 88, 82-103.
Knuth, D. (1968): The Art of Conlputer Progra,"nl;ny, Vois. 1- 3. Addison- Wesley,
New York.
Knuth, D. and Greene, E. (1982): Mathematics for the Anal)'s;s of Algorithm...
Birkhauser. Boston. MA.
Knuth, D., Graham, R.. and Patashnic, O. (1989): ("onerete Mathematic...: A f-oundatio'l
for Conlputer Science. Addison-Wesley, New York.
Kohn. R. (1984): 1'he ,nethod of partial regularity as applied to the Narier- Stokes
equal ions. I n: Chern, S. [ed.] ( 1984). pp. 117 - 128.
Kolomy, J. (1976): Some properties of monotone (potential) operators. Beitdige Anal. 8,
77-84.
Kolomy, J. (1978): Some nJethods for finding eigenvalues and e;gent'eClors of linear and
nonlinear operators. In: Kluge, R. [ed.], Theory of Nonlinear Operators, Akademie-
Verlag, Berlin.
Komura, Y. (1967): Nonlinear semigroups in Hilbert space. J. Math. Soc. Japan 19,
493- 507.
Kondratjev, V. (1967): Boundary l'alue problems for elliptic equations on domains ,,'ith
conical or angular points. Trudy Moskov. Mat. Obscestva 16, 209-292 (Rssian).
Kondratjev, V. and Olejnik. O. (1983): Boundary value problems .for partial differential
equations in nonsmooth domains. Uspekhi Mat. Nauk 38(2), 3 76 (Russian).
Konig, H. (1985): Eigenl'lIlue Distrihutions of Compact Operators. Birkhauser. Boston.
Konopelchenko. B. (1987): Nonlinear Integrahle Equations. Springer- Verlag. New
York.

1142
References
Koshclev, A. and Chelkak, S. (1985): Regularity of Solutiolls of Quasilinear Elliptic
Sy...tem.... Teubner, Leipzig.
Koshelev, A. (1986): Regularity of Solutions of Quasilinear Elliptic Systems. Nauka,
Moscow (Russian).
Kothe, G. (1969): Topological Vector Spaces, V ols. I, 2. Springer- Verlag, New York.
Krasnoselskii, M. (1956): Topological Melhod. in the Theory of Nonlinear Integral
Equations. Gostehizdat, Moscow (Russian). (English edition: McMillan, New
York, 1964).
K rasnoselskii, M. £It al. (1966): Integral Operators in Spaces of Summable Functions.
Nauka, Moscow (Russian). (English edition: NoordhofT, Leyden, 1976.)
K rasnosclsk ii, M. et al. ( 1973): N iiherungsverfahren zur 'Josullg von 0 peratorgleichun-
yen. Akadcmie- Verlag, Berlin.
Krasnoselskii, M. and Zabreiko, P. (1975): Geometrical Methods in Nonlillear Analysis.
Nauka, Moscow (Russian). (English edition: Springer- Verlag, New York, 1984.)
Krauss, E. (1985): A representation of maximal monotone operators b}' saddle functiolls.
Rev. Roumaine Math. Pures Appl. 30, 823 - 837.
Krauss. E. (19H5a): On the range of maximal monotone operators in flonrej1ex;(,e Banach
spaces. Math. Nachr. 120, 195 201.
Krauss, E. (1985b): A representation of arbitrary maximal monotone operators via
subgradients of skew-symmetric saddle functions. Nonlinear Anal. 9, 1381- 1399.
Krauss, E. (1986): Maxilnal monotone operators and saddle jUlictio1l..4i. Z. Anal.
Anwendungen 5, 333-346.
Krauss, E. (1988): On the max;nJality o.f the sum of monotone operators (to appear).
Krein, S. (1967): Linear Differential Equations in Banac'h Spaces. Nauka, Moscow
(Russian).
Krein, S. (1982): '#inear Equations in Banach spaces. Birkhauser, Basel.
Krein, S.. Petunin, E., and Semenov, E. (1982): Interpolat;oll of Li'lear Operators. Amer.
Math. Soc., Providence, RI.
Kruzkov, S. and Faminskii, A. (1985): Generalized solutions of the Cauchy problem for
the Korteweg-de Vries equation. Math. USSR-Sbornik 48, 391-421.
Krylov, N. (1985): Nonlinear Elliptic and Parabo/i,. Equations of Second Order. Nauka,
Moscow (Russian). (English edition: Reidel. Boston, MA, 1987.)
K ufner, A., John, 0., and Fucik, S. (1977): function Spaces. Academia, Prague.
Kufner, A. (1985): Weighted Sobolel' Spaces. Wiley, New York.
K ufncr, A. and Sandig, A. (1987): Some Applications of Weighted Sobolev Spaces.
Teubner. Leipzig. .
K urpcl, N. (1976): Projection-Iterative Method for Solution of Operator Equations.
Amer. Math. Soc., Providence, RI.
K uttler, J. and Sigilito, V. (1987): Estimating Eigenvalues with A Posteriori/ A Prior;
Inequalities. Pitman, London.
Ladde, G., Lakshmikantham, V.. and Vabala, A. ( 1985): Monotone Iterative 1ec:hniques
je}r Nonlinear Differential Equations. Pitman, London.
Ladyzenskaja, o. (1953): On the Mixed Problem for Hyperbolic Differential Equations.
Gostehizdat, Moscow.

References
1143
Ladyzenskaja. O. and Visik, M. (1956): Boundar)'-l\alue problems .for partial differ('ntial
equatiolls alld a class o.f operator equatiol1s. Uspekhi Mat. Nauk II (6), 41 -97
(Russian).
Ladyzenskaja. O. and Uralceva, N. (1964): Linear and Quasilinear Elliptic Equation....
Nauka, Moscow. (English edition: Academic Press, Nc\\' York, 1968; Second
. enlarged Russian edition, 1973.)
Ladyienskaja. 0.. Solonnikov, V.. and Uralceva, N. (1967): /..illear and Quasi/inedr
Parabolic Equations. Nauka, Moscow (Russian).
LadY1.enskaja, O. (1973): Tile BOUI1dar}'- Value Prohlems of Mathematical Ph}'sics.
Nauka. Moscow (R ussian). (Revised English edition: Springer- Verlag, 1985.)
Ladyi.enskaJa, O. and lJralceva, N. (1973): Second enlarged edition of Ladyzenskaja
O. and Uralceva, N. (1964).
Ladyzenskaja, O. (1985): Revised English edition of Ladyzenskaja. O. (1973). Springer-
Verlag, New York.
LadY7enskaja. O. and Uralceva, N. (1986): A surrey on tire solt'abilit)' of unfor'nly
elliptic and parabolic quasi/inear equat;ollS of se('ond order hal';lIg singularities.
Uspekhl Mat. Nauk 41 (5), 59- 83.
LadYl.enskaja. O. (1986): On some directions (r tire research in nrathematical phy.'iics al
the Steklol' Instilute in Leningrad. In: Vladimirov, V. [ed.] (1986), pp. 217-245
(Russian).
LadY1.enskaja. O. (1987): On the construcl;OIl of minimal glohal attractors .lor tile
Nat'ier-Stokes equations and other partial differential equat;ol's. Uspekhl Mat.
Nauk 42 (6). 25 - 60.
Lagrange. J. (1762): Essai d.u"e nout'elle methode pour deterlniner les maxima et minima
des formules integrates i'1de!inies. I n: Lagrange, J. (186 7). Vol. I. pp. 335- 362.
Lagrange. J. (1867): Oeut'res (Collected Jt()rks), V ols. 1 - 14. Paris, 1867 1892.
Landau. L. and l..ifsic, E. ( 1962): Course of Theoretical Phrs;cs, V ols. 1 10. Pergamon,
Oxford. (German edition, Akademie. Verlag, Berlin, 1962.)
Landes, R. (1985): Quasilinear h.rperholic variational inequalities. Arch. Rational Mech.
Anal. 91. 267- 282.
Landesman. E. and Lazer, A. (1969): Nonlinear perturbations of linear elliptic: houIJdar.r
value problems ar resonance. J. Math. Mech. 19. 609-623.
Langenbach, A. (1959): J1ariationsmethoden in der nichtlinearen Ela.'itiziliits- und
Plasliziliitstheorie. Wiss. Z. Humboldt-Univ. Berlin Math..Nat. Reihe 9. 145-
164.
Langenbach, A. (1965): J1erallgemei"erte und e_'(akte Losungen de. Prohlt'm.'i der
elasli.'ifh-plastischen Torsioll VOl' Stiiben. Math. Nachr. 28. 219 234.
Langenbach, A. (1966): 0 ber Gleichungen nrit Potentialoperatoren und M ;n;nlllrolgen
nichtquadratisclJer f'unktionale. Math. Nachr. 32, 9 - 24.
Langenbach, A. (1976): Monotone Potentialoperatoren in Theorie und An"'endungen.
VerI. der Wiss., Berlin.
Langenbach. A. (1984): Vorlesungen zur Ilijheren Analysis. Veri. der Wiss., Berlin.
Laplace. P. (1782): Theorie des attractio". des spheroides el de lafigure des planete.. In:
Laplace, P. ( 1878), Vol. 10. pp. 341-419.
Laplace. P. ( 1878): Oeul'res (C ol/ected 'orks), V ols. 1 - 14. Paris. 1878 1912.
Lasota. A. and Yorke, J. (1973): 1'he generic property of existence of ...olutions o.f
differential equat;olls on Barrach spaces. J. Differential Equations 13, 1- 12.
Laurent, P. (1972): Approximation et optim;sation. Hermann, Paris.

1144
References
Lax, P. (1953): 011 the equivalent g e between stability and convergence of difference
nrethods. Lecture held in a seminar at New York University. cr. Richtmyer, R. and
Morton, K. (1967), Chapter 3.
Lax, P. and Milgram, N. (1954): Parabolic Equatiolls. Annals or Mathematics Studies,
Vo.l. 33, pp. 167-190, University Press, Princeton, NJ.
Lax, P. and Richtmyer, R. (1956): Surt.'e.v 0.( the stahi/it y of linear finite difference
equat;o"s. Comm. Pure Appl. Math. 19. 437 -492.
Lax, P. (1964): Del 1 elopment of singularities of solutions of nonlinear h.vperbolic: equa-
tiolls. J. Math. Phys. 5, 611-613.
Lax, P. and WendrofT, B. (1964): Difference schemes for hyperbolic systems with high
order accuracy. Comm. Pure Appl. Math. 17,381 395.
Lax. P. and Nirenberg, L. (1966): On the stabilit}' of difference schemes: a sharp form
oj' Gdrding's i"equality. Comm. Pure Math. 19, 473- 492.
Lax, P. (1968): Integrals of no"linear equations of evolution a"d solitary wal'es. Comm.
Pure Appl. Math. 21,467 490.
Lax, P. (1973): Hyperbolic System.. of Conservation Laws and the Mathematical Theory
of Shock Waves. SIAM, Philadelphia, PA.
I.ax, P. (1983): Problems solved a"d u'Jsolved concerning linear a"d nonlinear partial
differential equatiolls. In: Warsaw (1983), Vol. I, pp. 119- 138.
Lax, P. (1984): Shock )VaVfS, i'lcrease 0.1" elltropy. and loss oj informatioll. In: Chern, S.
[ ed.] (1984), pp. 129 - 1 71.
Lebeau, G. (1986): Interaction des singularites pour les eqllations aux derivees partielles
'Ion lineuires d'apres J. Bony. Asterisque 133/34, 209 222.
Lebesgue, H. (1902): Integrale, lotlyeure, aire. Annali Mat. Pura Appl. 7, 231- 359.
Lee, T. (1981): Particle Ph}'sics and Introduction to Field Theory. Harwood, New York.
Lee, J. and Parker, T. (1987): The Ya,,,ahe problem. Bull. Amer. Math. Soc. (N.S.) I',
37 -91.
Legendi, T. and Szentivanyi, T. (1983): leben und Werk des John von Neumann.
Bibliograph. In51., Mannheim.
Legendre, A. (1786): cr. Stackel, P. [ed.] (1894).
Leis, R. (1986): 1,lit;al-Bou,ldary Value Problems i'l Mathematical Physics. Wiley, New
York.
Leray, J. (1934): E.'isa; sur les mouvements plans d'une liquide v;sceu..'( que Iinlite,1t des
parao;s. J. Math. Pures Appl. 13, 331- 418.
Leray, J. (1952): II }'perholic Differential Equatio'is. Institute for Advanced Studies,
Princeton, NJ.
Leray, J. and Lions. J. (1965): Quelques resultats de Visik sur le. problemes elliptiques
non linea;res par les methodes de Mint }'- Browder. Bull. Soc. Math. France 93,
97 107.
Leray, J. and Ohya, Y. (1967): Equations et syslemes non linea;res hyperbolic non-:.lri('l.
Math. Ann. 170, 167 205.
Levitan. B. (1984): The Inverse Sturm Lioul1ille Problem. Nauka, Moscow (Russian).
Lichnerowicz, A. (1967): Relativistic Hydrodynamics and Magnetohydrodynamics.
Benjamin, New York.
Lichnewsky, A. and Saguez, C. [eds.] (1987): Superco,nputing: the Slale of ,he Art.
North-Holland, Amsterdam.
Lichtenstein, L. (1921): Nellere Entwi(Oklung der Potentialtheurie. In: Encyklopiidie der
Mat henltlt ;.che'l Wissenschtifte'l ( 1 899fT), Vol. 2, Part 3. L pp. 181 - 377.

References
1145
Lieberman, G. and Trudinger, S. (1986): Nonlinear oblique boundary I'alue prohlems for
1J0niinear elliptic' equations. Trans. Amer. Math. Soc. 195, 509 - 546.
Linl-. P. (1988): .4 critique of nunJer;cal analysis. Bull. Amer. Math. Soc. (N.S.) 19,
407 416.
Lions, J. (1955): Prohlenles au. lim;tes en tl't)or;e des distributions. Acta Math. 94,
13- 153.
Lions, J. (1959): Theoremes dt' traces et d'interpolatioll, I V; I-II. Ann. Scuola Norm.
Sup Pisa ("I. Sci. 13(1959),389-403,14(1960),317 331; III.J. Math. Pures AppJ.
42 (1963),196-203; IV, Math. Ann. 151 (1963),42- 56; V. Anais de Acad. Brasileira
de Ciecias 35 ( 1963), 1 - 110.
Lions. J. and Magenes. E. (1960): Prohlenles al4X Ii",ites non Itomoyenes, 1- VII; I. III,
IV, V, Ann. Scuola Norm. Sup. Pisa CI. Sci. 14(1960),259-308; 15(1961),39-101;
311 326; 16 (1962), 1 44, II. Ann. Inst. Fourier II (1961), 137 178; VI, J. Analyse
Math. II (1963).165-188; VII, Annali Mat. Pura Appl. 4, LXIII (1963). 201-224.
Lions, J. (1961): Equal ivns differellt ielles operat i()lIelles et prohlemes aU.t linr;t es.
Springer- Verlag, Berlin.
Lions. J. and Stampacchia, G. (1967): Variational inequalities. Comm. Pure Appl.
Math. 20,493- 519.
Lions, J. ( 1968): C ont role opt imal des syst emes gOIlI'ernes par des equat ions aux derifees
partielles. Dunod, Paris. (English edition: Springer- Verlag, Berlin, 1971.)
Lions, J. and Magenes, E. (1968): Prohle,nes uu. Iinrites non homogenes el applIcations,
V ols. I - 3. Dunod, Paris. (English edition: Springer- Verlag, Berlin. 1972.)
Lions, J. (1969): Quelql,es methodes de resolution des problenJes aux lim;tes non linea;res.
Dunod, Paris.
Lions, J. and Dautray, R. (1984): Analyse matlJemalique ('t calcul 11ulnerique pour les
sciencl)s et les techllique. V ols. I - 3. Masson, Paris.
Lions, J. and Ciarlet, P. [eds.] (1988): Handbook of Numerical Analysis, Vols. I, 2.
Vol I : Finite Element Method. Vol. 2: f.;nite Difference Method (Vols. 3fT to appear).
North-Holland, Amsterdam.
Lions, P. (1982): On the e_istt'nce of posit;l'e solutions of semilinear elliptic equations.
SIAM Rev. 24,441-467.
Lions, P. (1983): Generalized Solutions of Ilamiiton-Jacohi Equations. Pitman,
London.
Lions, P. (1983a): Ilanliiton-Jacobi - BellnJan equations and the optimal conlrol oj
stochastic ..ystems. In. Warsaw (1983), Vol. 2,1403- 1417.
Lions, P. (1984): The concentration-t'ompactness principle in the calculus of variations.
Ann. Inst. H. Poincare. Anal. Non Lineaire I, 109- 145.
Lions, P. (1985): Sur les equations de Monge-Ampere. Arch. Rational Mech. Anal. 89,
93- 122.
Ljapunov, A. (1906): Sur It's figures d'equi/ihre. In: Ljapunov, A. ( 1959), Vol. 4, 225 pp.
Ljapunov, A. (1959): Collected Works, Vols. 1-5. Academy of Sciences, Moscow
(R ussian).
Majda, A. (1984): Compressible Fluid Flo\\' and Systems vf Conser[ati()11 La\\'s in Sereral
Space Variables. Springer- Verlag, New York.
Malgrange, B. (1955): E.;stence et appro,-,:imation des solutions des equations aux
der;l'ees part;elles et des equations de conl'olution. Ann. Inst. Fourier 6, 271 - 355.

1146
References
Mandelbrot, B. (1982): The Fractal Geometry of Nature. Freeman, San Francisco, CA.
Marchenko, V. (1988): Nonlinear Equations and Operator Algebras. Reidel, Boston,
MA.
Marchuk, G. and Shaidurov, V. (1983): Difference Method,'} and Their Extrapolations.
Springer- Verlag, New York.
Marsden, J. and Hughes, T. (1983): Mathematical Foundations of Elastic;t}'. Prentice-
Hall, Englewood Cliffs, NJ.
Martin, R. (1976): Nonlinear Operators and Differential Equations in Banach Spaces.
Wiley, New York.
Martin. J. [ed.] (1988): Performance Evaluation of Supercomputers. North-Holland.
Amsterdam.
Maslennikova, V. (1988): Lf-theory for elliptic boundary value problems with nonsmooth
alld 1I0llCOnJpact boundary (to appear).
Maso, G. and Mosco, U. (1986): Wiener criteria and energy decay for relaxed Dirichlet
problems. Arch. Rational Mech. Anal. 95, 345-387.
Masuda, K. and Suzuki, T. (1988): Recent Topics in Nonlinear PDEs III. North-
Holland, Amsterdam.
Mathematics: 1°he VIlifying 1"hread ill Science (1986): Notices of the American Mathe-
matical Society, Vol. 33. pp. 716-733.
Matveev, V. et al. (1986): Algebraic-geometrical principles of the superposition of finite-
gap solutions of integrable nonlinear equations. Uspekhi Mat. Nauk 41 (2), 3-42
(R ussian).
Maurin. K. (1967): Methods of Hilbert Spa('es. P\\'N, Warsaw.
Maurin, K. (t 982): Plato's cave parable and the development of modern phys;('s. Rend.
Sem. Mat. Univ. Politec. Torino 40, 1- 31.
Mawhin, J. (1972): Equivalence theorems for nonlinear operator equations and coin-
cidence degree for some mappin!}s in locally convex spaces. J. Differential Equations
12,610-636.
Mawhin, J. (1979): Topological Degree Methuds in Nonlinear Boundary Value Problems.
Amer. Math. Soc., Providence, RI.
Mawhin, J. (1981): Cf. the Preface to Fucik, S. (1981).
Mawhin, J. (1986): Variational methods and se,nilinear elliptic equations. Arch. Rational
Mech. Anal. 95, 269-- 277.
Mawhin, J. (1986a): Necessary and sufficient conditions for the solvability of nonlinear
eqllations through the dual least action principle. In: International Workshop on
Applied Differential Equations, Beijing, pp. 91-1 08. World Scientific, Singapore.
Mazja, V. (1985): Sobolev Spaces. Springer-Verlag, New York.
Mazur, S. (1933): Ober konvexe Mellgen in linearen normierten RiJumen. Studia Math.
5, 70 84.
Meis, T. and Marcowitz, U. (1978): Numerische Behandlung partie/ler Differential-
gleichungen. Springer- Verlag, Berlin. (English edition: Numerical Solutions of
Partial Differential Equations. Springer-Verlag, New York, 1981.)
Michlin, S. (1962): Variationsmelhoden der mathematischen Ph.vsik. Akademie- Verlag,
Berlin.
Michlin, S. (1969): Nunlerische Realisierung von Variationsmethoden. Akademie- Verlag,
Berlin. (English edition: Cr. Mikhlin, S. (1971 )).
Michlin, S. and Smolickii, C. (1969): N allerungsmethoden zur Losung von Differential-
ulld Integralgleichungen. Teubner, Leipzig. (English edition: Mikhlin, S. and
Smolitskiy, K., Approximate Methods for Solution of Differential and Integral
Equations. American Elsevier, New York, 1967.)

References
1147
Michlin. S. (1975): Lehrgang der mathematischen Physik. Akademie- Verlag, Berlin.
Mlchlin. S. (1976): Approximation auf dem kubischen Gitter. Akademie- Verlag, Berlin.
M ichlin. S. and ProBdorf, S. (1980): Singuliire I ntegralgleichungen. Akademie- Verlag,
Berlin. (English edition: Singular Integral Equations, Berlin. 1982.)
Michhn. S. (1981): Konstatuen in einigen Unyleichungen der Analysis. Teubner. Leipzig.
(English edition: Mikhlin, S., Constants in Some Inequalities of Anal}'sis. Wiley,
New York, 1986.)
M ichlin. S. (1985): F ehler in numerischen Prozessen. Akademie- Verlag, Berlin.
M iersemann, E. (1982): Zur Regularitiit verallgemeinerter LOsungen von quasi/inearen
elliptischen Differen,;algleichungen zM.eiter Ordnung in Gebieten mit Ecken. Z. Anal.
Anwendungen I (4), 59- 71.
Miersemann. E. (1988): Asymptotic expansions of solutions of the Dirichlet prohlem .for
quasi-linear elliptic equations of second order near a conical point. Math. Nachr.
135. 239-274.
Micrsemann, E. (1988a): Asymptotic expansions at a corner for the capillary prohlem.
Pacific J. Math. 134, 299- 311.
M ihailov, A., Sabat, A., and Jamilov, R. (1987): A symmetric approach to the flassifi-
(.ution of nonlinear equations: A full classification of integrahle s}..tems. lJspekhi
Mat. Nauk 42 (4),3-53 (Russian).
Mikhlin, S. (1971): The Numerical Performance (r Variational Methods. NoordhofT,
Groningen.
Minty. G. (1962): Alonotone operator. in IIUbert spaces. Duke Math. J. 29,341-346.
Minty, G. (1963): On a monotonicit}' method for the solution of non-linear equatio'Js in
Banach spaces. Proc. Nat. Acad. Sci. U.S.A. SO. 1038-1041.
Minty. G. (1978): On (.ariational inequalities for monotone operators. Adv. in Math. JO,
1-7.
Miranda, C. (1970): Partial Differential Equatio'is of Elliptic Type. Springer- Verla
Berlin.
Miranker, W. (1981): Numerical Methods for Stiff Equatio,r.-. and Singular Perturbation
Prohlems. Reidel. Boston.
Morawetz. C. [ed.] (1986): Frontiers of the Mathematical Sciences. Comm. Pure Appl.
Math. 39, Supplement S. The entire issue.
Morrey, C. (1940): Existence and differentiahility theorems for the solutions of (\aria-
tional problems for multiple integrals. Bull. Amer. Math. Soc. 46. 439 458.
Morrey, C. (1966): Multiple I ntegrals in t he Calculus of Variations. Spnnger- Verlag,
Berlin.
Morse, P. and Feshbach, H. (1953): Methods of Theoretical Physics, Vois. I, 2.
McGraw-Hili, New York.
Mosco, U. (1973): An Introduct;o,J to the Appro.'-:;mate Solution of Variational
Inequalities. Ed. Cremonese. Roma.
Moser, J. (1961): On Harna(.k's theorem for elliptic differe,Jt;al equations. Comm. Pure
Appl. Math. 14. 577 - 591.
Moser, J. (1966): A rapidly convergent iteration method and nonlinear partial differential
equations. Ann. Scuola Norm. Sup. Pisa CI. Sci. 20. 265- 315; 499- 535.
Moser, J. (1981): Integrable Hanliltonian Systems and Spectral Theory. Lezione
J."ermiane, Pisa.
Moser, J. (1986): Minimal .-.olutions of IJariat;onal problems on a torus. Ann. Inst. H.
Poincare. Anal. Non Lineaire 3, 229-272.
M ukherjea, A. and Pothoven, K. (1978): Real and FunctiotJal Analysi.c;. Plenum, New
York.

1148
References
M tiller, M. (1974): Das DijJerenzenL'erjahren fUr quasi/illeare ellipt ische Differe,u ial-
gleichunyel' hoherer Ordnuny. Math. Nachr. 63, 255 275.
Mumford, D. (1983): Tata Lectures on T/.eta. Birkhauser, Boston, MA.
Murat, F. (1987): A survey of compensated compa('tness. In: Cesari, L. [ed.] (1987),
pp. 145 - 183.
Murman, E. [ed.] (1985): Progress and Supercomputing i" Computational Fluid
Dylla".;c.... Birkhauser, Boston.
Myint, U. and Debnath, L. (1987): Partial Differe,uial Equations for Scientists and
Elzyil1eers. North-Holland, New York.
Nagel, R. et al. (1986): One-Parameter Linear Semigroups of Positive Operators.
Springer Lecture Notes in Mathematics, Vol. 1184. Springer-Verlag, Berlin.
Nash, J. (1958): Continuity of solutiuns of parabolic alJd ellipti(O equations. Amer. J.
Math. 80, 931- 954.
Natanson, I. (1955): Konstruktive Funktionentheorie. Akademie- Verlag, Berlin.
Necas, J. (1967): us n.etlJodes directes en theorie des equations elliptiques. Academia,
Prague.
Necas, J. (1973): On the ra"yes of nOlJ/inear operators with linear as}'mptotes \vh;ch are
1101 ;lJvertihle. Comment. Math. Univ. Carolin. 14, 63 72.
Necas, J. (1977): Example of all irregular solution to a nonlinear elliptic systeln v.'ith
analytic coefficients and conditions .fur regularity. Abh. Akad. Wiss. DDR, Mathe-
matik I, 197 - 206.
Necas, J. and Iflavacek, I. (1981): Mathelnatical Theory of Elastic and Elasto-Plastic
Bodie.... Elsevier, Amsterdam.
Necas, J. (1982): The solutiolJ of the 19th Hilhert problem. In: Kurke, H. et al. [eds.]
(1982), Recent 1°rellds in 4\1 athelnati(:s, pp. 214 223. Teubner, Leipzig.
Necas, J. (1983): Introduction to the Theory of Nonlinear Elliptic Equations. Teubner,
Leiplig.
Neumann, J. v. (1929): Allgemeine Eigenwerttheorie hermitescher Funktionaloperatoren.
Math. Ann. 102, 49-131.
Neumann, J. v. (1929a): Zur rrheorie der uI.beschriinktell Matrizen. J. Reine Angew.
Math. 161, 208 - 236.
Neumann, J. V. (1932): Mathematische Grundlayen der Quantenmechanik. Springer-
Verlag, Berlin. (English edition: Mathematical ""oundations of Quantum Me(:hanics,
University Press, Princeton, NJ, 1955.)
Neumann, J. v. and Richtmyer, R. (1947): On the "umerical solution of partial differential
equat;o"s of parabolic type. In: Neumann, J. V. (1961), Vol. 5, pp. 652-663.
Neumann, J. v. (1949): On rings of operators. Ann. Math. SO, 401- 485.
Neumann, J. v. (1961): Collected Works, Vols. 1-6. Pergamon, New York.
Newell, A. el ul. (1987): Soliton Mathematics. Les Presses de I'Universite de Montreal.
NI, W. and Serrin, J. (1985): Nonexistence theorems for partial differential equations.
Rend. Cire. Mat. Palermo (2), Suppl. 8, 171-185.
Nicolaenko, B. et £II. [ed.] (1986): Nunlinear S}'stems of PDEs in Applied Mathematics,
Parts I, II. Amer. Math. Soc., Providence, RI.
Nikolskii, S. (1975): Approximation of FUllct;ons of Several Variables and Embedding
7'heorems. Springer- Verlag, New York.
Nirenberg, L. (1955): Relnarks on strongly elliptic differential equations. Comm. Pure
Appl. Math. 8,649-675.

References
1149
Nirenberg, L. (1959): On elliptic partial differential equations. Ann. Scuola Norm. Sup.
Pisa ('1. Sci. 13, I - 48.
Nirenberg. t. (1966): An ('.'(tended i,uerpolation inequality. Ann Scuola Norm. Sup.
Pisa CI. Sci. 20, 733- 737.
Nirenberg. L. (1970): All applicatio'l (r generalized degree to a class of nonlinear
problems. Troisieme Colloq. Analyse, Liege, Math. Vander. pp. 57 74.
Nirenberg, L. (1972): An ahstract form of the nonlinear Cauchy- Kovalet'skaJa theorem.
J. Differential Geom. 6" 561- 576.
Nirenberg. L. (1974): Topics in Nonlinear Functional AtJalysis. Courant Institute, New
York U ni\'ersity.
Nirenberg, L. (1979): Cf. Gidas, 8. et ale (1979).
Nirenberg, L. (1981): Variational and topological methods in nonlinear problem. Bull.
Amer. Math. Soc. (N.S.) 4, 267- 302.
Nirenberg, L. (1984/88): Cf. Caffarelli, l. et al. (1984/88).
Nishida, T. (1977): 0'1 a theorem of Nirenberg. J. Differential Geom. 12, 629 633.
Nitsche, J. (1970): Lineare Spline-Fllnktionen und die Methode von Ritz fUr elliptische
Rand,vertprohleme. Arch. Rational Mech. Anal. 36, 348- 355.
Nohel, J., Hrusa, W., and Renardy, M. (1987): A1athematical Problems in Visco-
elasticit y. Wiley, New York.
Nohel. J.. Rogers, R., and Tzavaras. A. (1988): K'eak solutions for a nonlinear s)'stenl in
l';scoela.'tt;cit y. Comm. Partial Differential Equations 13, 97 - 127.
No\'ikov. S. et al. (1980): Theory of Solitons. Nauka, Moscow (Russian). (English
edition: Plenum, New York. 1984.)
Oberguggenberger, M. (1986): Propagation of singularities for semilinear hyperbolic
initial-houndary l'alue problems in one space dimension. J. Differential Equations
61. I 39.
Oberguggenberger. M. ( 1987): Generalized solut ions to semilinear hyperbolic systems in
tK'O r:ariables. Monatsh. Math. 103, 133-144.
Oberguggenberger, M. (1988): Weak limits of solutions to semilinear hyperbolic systems.
Math. Ann. (to appear).
Oleinik, o. (1957): Discontinuous solutions of nonlinear differential equation.... Uspekhi
Mat. Nauk 12 (3), 3- 73 (Russian).
Olejnik, o. and Kruzkov, S. (1961): Quas;linear parabolic equations of second order.
Uspekhi Mat. Nauk 16 (5), 115 -155 (Russian).
Olejnik, O. et al. (1979): Homogenization and G-conl'ergence of differential operators.
Uspekhi Mat. Nauk J4 (5 65-133 (Russian).
Oleinik, O. and Kondratjev, V. (1983): Boundary t,alue prohlems for partial differential
equations in nonsmooth domains. Uspekhi Mat. Nauk 38 (2), 3-76 (Russian).
Osborn, J. (1975): Spectral approximation for compact operators. Math. Compo 29,
712 725.
Osserman, R. (1986): A Surl'ey of Minimal Surfaces. Dover, New York.
Ostrowski. A. (1951): Vorlesungen uher Differential- und Integralrechnung, V ols. I - 3.
Birkhauser, Basel.
Otte, M. [ed.] (1974): Mathematiker ubr Mathematik. Springer- Verlag, Berlin.
Ovsjannikov, L. (1976): Cauchy problem in a scale of Banach spaces and it. application
to the shalloK' ,,'aler justification. In: Germain. P. and Nayroles. B. [eds.] (1976),
pp.426--437.

1150
References
Pao, C. (1978): Asynlplotic behavior and nonexistence of glohal solutions for a class of
no,Jiinear boundary value problems of parabolic type. J. Math. Anal. Appl. 65,
616-637.
Pao, C. (1979): Bifurcati011 analysis on a 110nlinear diffusioll sy.-.tem in reactor dynamics.
Applicable Anal. 9, 107-119.
Pao, C. (1980): Nonexistence of global solutions for an integro-differential system in
reactor dynamics. SIAM J. Math. Anal. 11,559-564.
Pascali, D. and Sburlan, S. (1978): Nonlinear Mappings of Monotone Type. Academiei,
Bucuresti.
Pavel, N. (19M7): N01a/inear Evolution Operators and Semigroups. Springer- Verlag, New
York.
Payne, L. (1976): Improperly Posed Problems in Partial Differential Equations. SIAM,
Philadelphia, P A.
Pazy, A. (1983): Semigroups of Li'lear Operators and Applications to Partial Differential
Equations. Springer-Verlag, New York.
Peitgen, H., Saupe, D., and Schmitt, K. (1981 ): Nonlinear elliptic boundary value
problenJs versus their finite difference approximations: numerically irrelevant solu-
tions. J. Reine Angew. Math. 322, 74-117.
Pctryshyn, W. (1967): Iterative constru(otion of fixed points of contractive type mappings
in Banach .-'paces. In: Numerical Analysis of Partial Differential Equations, pp.
307 - 339. C.J.M.E., Edizione Cremonese, Roma.
Petryshyn, W. (1968): On the approximatioll-solvability of n0111i'lear equations. Math.
Ann. 177, 156-164.
Petryshyn, W. (1970): Nonlinear equations involving nO'lcompact operators. In: Browder,
F. [cd.] (I 97Oc), pp. 206- 233.
Petryshyn, W. (19703): A characterization of strift cont'exity of Banach spaces and other
uses of duality mappings. J. Funct. Anal. 6, 282 - 291.
Petryshyn, W. (1975): On the approximation-solvability of equatio'is involving A-proper
and pseudo-A-proper mappings. Bull. Amer. Math. Soc. 81, 223 - 312.
Petryshyn, W. (1976): On the relationship of A-properness to mappings of monotone type
with applications to elliptic equations. In: Swaminatha, S. [ed.] (1976): I:ixed-Point
Theory and Its Applications, pp. 149-174. Academic Press, New York.
Petryshyn, W. (1980): Using degree theory for densely defined A-proper maps in the
solvability of semilinear equations with hounded and noninl'ertible linear part.
Nonli near Anal. 4, 259 - 281.
Petryshyn, W. (1980a): Solvability of linear and quasilinear elliptic boundary value
problems via the A-proper nlapping theory. Numer. Funct. Anal. Optim. 2, 591 -635.
Petryshyn, W. (1981): Variational solvability of quasilinear elliptic boundary value
problems at resonance. Nonlinear Anal. 5, 1095-1 108.
Petryshyn, W. and Yu, Z. (1985): On the solvability of an equation describing the periodic
motions of a satellite in its elliptic orbit. Nonlinear Anal. 9. 969 -975.
Petryshyn, W. (1986): Approximation-solvability of periodic boundary value problems
via the A-proper mapping theory. Proc. Sympos. Pure Math. Vol. 45, pp. 261- 282,
Amer. Math. Soc., Providence, RI.
Petryshyn, W. (1986a): Solvabilit}, of various boundary value problems for the equation
x" = f(t,x,x',x") - y. Pacific J. Math. 122, 169-195.
Petryshyn, W. (1987): Existence of positive eigenvectors and fixed pOi11tS for A-proper
type maps in cones. In: Liu, 8. [ed.] (1987), Proceedings of the International
Conference in Honor of Ky Fan. Dekker, New York. pp. 59-63.

References
1151
Petryshyn. W. (1987a): Multiple pos;t;I'e fixed points of ,nult;ral'4ed condensing map-
pings 'ith some applications. J. Math. Anal. Appl. 124,237-253.
Piessens, R. et al. (1983): QUADPACK: A Subroutine Package for Automatic Integra-
tion. Springer- Verlag, New York.
Pietsch, A. (1987): Eige,u;alues and s-Numhers. Geest & Portig, Leipzig.
Piran. T. and Weinberg, S. (1988): Strings and Superstrings. World Scientific, Singapore.
PissanetLky. S. (1986): Sparse Matrix Technology. Academic Press. New York.
Pogorzelski. W. (1966): Integral Equations and Their Applications. PWN, Warsaw.
Pohoiaev, S. (1965): On eigenfunctions of the equation - Au = ;f(u). Doklady Akad.
Nauk SSSR 165 (I), 36- 39 (Russian).
Pohoiaev, S. (1967): On the so/r'ahi/ity of nonlinear equations involt\ing odd operators.
Funktional. Anal. i Prilozen. I (3), 66 - 73 (Russian).
Pohol11ev, S. (1969): On nonlinear operators 'ith weakly closed range and quasi-linear
elliptic equations. Mat. Sbornik 78, 237 259 (Russian).
Poincare, H. (1890): Sur les equations au): deri('ees partielles de la physique nlathe-
nlatique. Amer. J. Math. 11,211-294.
Poincare, H. (1916): OeUL'res (Collected Works), Vols. 1-11. Gauthier-Villars, Paris.
Poschel, J. and Trubowitl, E. (1987): Inverse Spectral Theory. Academic Press, New
York.
Press.'''. et al. (1986): Numerical Re,.ipes and Computer Programs: The Art of Scientific
Computing. University Press, Cambridge, England.
Prol3dorf, S. and Silbermann, B. (1977): Projektion.'il'erfahren und die niiherungs'e;se
Losung singuliirer Gle;chungen. Teubner, Leipzig.
Rabier, P. (1986): A general study 0.( nonlinear problems M-,;th three S01141ion... in H ilhert
spaces. Arch. Rational Mech. Anal. 95, 123-154.
Rahino\\'it1, P. (1974): Variational methods for nonlinear eigenl'alue problems. In: Prodi.
G. [ed.], Eigenvalues of Nonlinear Problems, pp. 141 195, Ed. ("remonese, Roma.
Rabinowit7, P. (1977): A bifurcation theorem for potential operators. J. f.unct. Anal. 26,
48 -67.
Rabinowit P. (1978): Free vibrations for a .emilinear wave equation. Comm. Pure
Appl. Math. 31.31-68.
Rabino\\'itz. P. (1984): Minimax methods and their applications to partial differential
equations. I n: Chern, S. [ed.] (1984), pp. 307 - 320.
Rabinowitz.. P. (1984a): Large amplitude time-periodic solutions of a semilinear K-'al'e
equation. Comm. Pure Appl. Math. 37, 189- 206.
Rabinowitz, P. (1986): Methods in Critical Point Theory 'ith Applicatio1Js to Differ-
ential Equations. Amer. Math. Soc., Providence, RI.
Rajaraman, R. (1982): Solitons and Instantons. North-Holland, Amsterdam.
Rannacher, R. (1980): On .finite element approximation of general boundar)' I'alue
pruhlems in nonlinear elasticit}'. Estratto da Calcolo 17, 174 -193.
Rauch, J. and Reed, M. (1980): Propagation of .ingularitjes for .'iemi/inear hyperbolic
equations in one space variable. Ann. Math. III, 531- 552.
Rauch, J. and Reed, M. (1981): Jump discontinuities of semi linear, strictly h.rperbolic
systems in two t'ariahles. Comm. Math. Phys. 81, 203-227.
Rauch, J. and Reed, M. (1982): Nonlinear microlocal analysis of semilinear hyperbolic
system... in O'I£, space dimension. Duke Math. J. 49. 397 -475.

1152
References
Rauch, J. and Reed, M. (1988): Nonlinear superposition antI ab!iOrbtion of delta waves
in olle space dimension. J. f.unct. Anal. (to appear).
Raviart, P. (1967): Sur fapproximatio" de certaines equations J'evolulion Iineaires. J.
Math. Pures Appl. 46, II - 183.
Rebbi, C. and Soliani, G. [eds.] (1984): Solitons and Partirles. World Scientific,
Singapore.
Reed, M. and Simon, B. (1971): Methods of Modern Mathematical Plrysic., Vols. 1 - 4.
Academic Press, 1971.
Reed, M. (1976): Abstract No,,/inear Wave Equations. Lecture Notes in Mathematics,
Vol. 507. Springer- Verlag, Berlin.
Reich, S. (1984): New results concerning accretive operators and nonlinear semigroups.
J. Math. Phys. Sci. 18,91-97.
Reid, C. ( 1970): Hilbert. Springer-Verlag, New Yark.
Reid, C. (1976): Courant ill Gottinge1l alld New York. Springer- Verla New Yark.
Reinhardt, II. (1985): A1Ial}'sis of Approximation Methods for Differential- alld Integral
Equations. Springer-Verlag, New York.
Rektorys, K. (1977): Variational Methods in Mathematics, Sciellce and Engineerillg.
Reidel, Boston, M A.
Rektorys. K. (1982): The Method of Discretization i,. Time and Partial Differential
Equal ions. Reidel, Boston, M A.
Rellich, F. (1930): Ein Satz uber mittlere KOIlt'ergenz. Nachr. Akad. Wiss. Gottingen,
pp. 30 35.
Rempel, S. and Schulze, B. (1982): Index Theory of Elliptic Boundary Value Problems.
Akademie- Verlag, Berlin.
Renardy, M., Hrusa, W., and Nohel, J. (1987): Malhenlatical Problems in Visco-
elasticity. Wiley, New York.
Rhodius, A. (1977): Der nllmerische Werlebereich und die l..osharkeit linearer ulld I.ichl-
Iillearer Operatorgleichungen. Math. Nachr. 79, 343-360.
Rice, J. (1983): Numerical Methods, Software, and Analysis. McGraw-Hili, New York.
Rice, J. and Boisvert, R. ( 1984): Solving Ellipt ic Problems V sing E Ll..P A C K. Springer-
Verlag, New York.
Richtmyer, R. and Morton, K. (1967): Differellce Methods for Initial- Value Prohlems.
Interscience, New York.
Riemann, 8. (1851): Grundlagen fUr eine allgemeine 1"heor;e der Funktionen einer
l'erullder/ichen ko,nplexen Grope. Dissertation. I n: Riemann, B. (1892, pp. 3 43.
Riemann, B. (1857): Theorie der Abelschen Funktionen. In: Riemann, B. (1892),
pp.88-142.
Riemann, B. (1892): Gesammelte mathematische Werke (Colle,.red Works), Vols. 1,2.
Leipzig, 1892. Nachtrage (Supplements), Leipzig, 1902.
Riesl, F. (1918): 0 ber lineare Funktionalgleichungen. Acta Math. 41, 71-98.
Riesz, F. (1934): Zur Theorie des Hilbertschen Raumes. Acta Sci. Math. (Szeged) 7,
34 38.
Riesz, F. and Nagy, B. (1956): Voriesunge'l uber Funktionalanalysis, VerI. der Wiss.,
Berlin. (English edition: f'unctional,4nalysis, Ungar, New York, 1955.)
Riesl, F. (1960): Oeuvres completes (Collected Works), Vols. 1-4. Hungarian Academy
of Sciences, Budapest.
Riesl, M. (1926): Sur les maxi,na des formes bi!ineaires et sur les f01lctionnelles linea ire....
Acta Math. 49,465-497.

References
1153
Ritz, W. (1909): ilber eine neue Methode zur IJosung gewisser Variationsprobleme der
mathematischen Ph}'sik. J. Reine Angew. Math. 135. 1-61.
Ritz, W. (l909a): Theorie der 7ransl'ersalsch,,';ngungell eiller quadratische" Platte ,nit
freit'IJ Riindern. Ann. Physik 18, 737 - 786.
Rjahenki, V. and f- 1 ilippov, A. (J 960): () ber die Slahi!itat "Oil Differell:ellyleichungen.
VerI. der Wiss., Berlin.
Rockafellar, R. (1966): Characterization o.f the sllhdifferential of conl'ex .functiolIS.
Pacific J. Math. 17,497-510. (Cr. also Rockafellar, R. (1970b).)
Rockafellar, R. (1968): C onl't'_'\: functions. montone operators. and I'ariational inequalities.
In: Proceedings of the NATO Advanced Study Institute, pp. 35 65. Ed. Oderisi,
Gubbio. Italy.
Rockafellar. R. (1969): lAIcal boundedness (f nonlinear monotone operators. Michigan
Math. J. 16. 397 -407.
Rockafellar. R. (1970): On the maximal monotonic;t.\' of SUIPIS of nOlJlinellr monotone
operators. Trans. Amer. Math. Soc. 149, 75 -88.
Rockafellar, R. ( 1970a): C onl'e:c Analysis. University Press. Princeton. NJ.
Rockafellar, R. (1970b): On the maximal mOllotonicity or subdifferelllial mappillgs.
Pacific J. Math. JJ, 209 - 216.
Rockafellar. R. (197Oc): ,.lonolone operators associated ,,'ith saddle-.functions and
",illima:( problt'"'s. In: Browder, F. [ed.] ( I 97Oc), pp. 241- 250.
Rockafellar. R. (197Od): On the I'irtual convexit}' of the range o.f a I.unlinear maximal
operator. Marh. Ann. 185. 81 - 90.
Rockafellar, R. (1985): Ma,imal monotone relations and the second deril'atil'es o.f
nOllsmooth functions. Ann. Inst. H. Poincare. Anal. Non Lineaire 2. 167 184.
Rosinger. E. (1987): Generali:ed Solutions (,. Nonlinear Partial Differe,u;al Equat;olJs.
North-Holland, Amsterdam.
Rothe. .... (1984): Global Solution... (r Reaction - Diffusion Syste",s. Springer- Verlag. New
York.
Rothe. E. (1986): Introduction to J'arious Aspects oJ' Degree .rheory i" Banach Spaces.
Amer. Math. Soc., Providence. RI.
Royden. H. (1988): Real Analysis. Macmillan. New York.
Roidestvenskii. B. and Janenko, N. (1978): Systems o.f Quasilinear Equations and 1'heir
Applications to Gas Dynamic.... Nauka Moscow. (English edition: Amer. Math.
Soc., Providen, R I, 1983.)
Ruf, B. (1986): Multiplicity results .for nonlinear elliptic equations. In Krbec. M. et al.
[ eds.]. Nonlinear Anal.vsis, pp. 109- 138, Teubner, Leipzig.
Runst, T. (1985): Paradifferential operators ill .paces of Triehel- Lizorkill and Besol'
lype. Z. Anal. Anwendungen 4, 537 - 573.
Sacks. J. and Uhlenbeck. K. (1981): The existence (f minimal immersions of 2-spheres.
Ann. Math. 113, 1- 24.
Sakamoto, R. (1982): Hyperbolic Boundary Vallie Problems. University Press. Cam-
bridge. England.
Samarskii, A. (1984): Theorie der Differellzenl'erfahren. Geesl & Portig. Leipzig.
Sard. A. (1942): The Ineasure of the critical points of differentiable maps. Bull. Amer.
Math. Soc. 48. 883 -890.
Sauer. R. (1958). Anfangs,,'ertprobleme be; partiellell Diffi'rentialg/(Jichuny()n. Springer-
Verlag, Berlin.

1154
References
Sauer, R. and Szabo, I. (1967): Mathenlat;sche Hi/f.fimittel des IngelJieurs, Vols. 1-4.
Springer- Verlag, Berlin.
Schauder, J. (1930): ()ber lineare vollstetige Funktionaloperationen. Studia Math. 1,
103- 196.
Schauder, J. (1935): Das Anfallgswertproblem einer quasilinearen hyperbolisfhen Diffe-
relu;algleichung zwe;ter Ordnung mit beliebig vielen uIJabhangigen Variablen. Fund.
Math. 14, 213- 246.
Schechter, M. (1971): Spectra of Partial Differential Operators. North-Holland,
Amsterdam.
Schechter, M. (1973): A nonlinear elliptic boundary value problem. Ann. Scuola Norm.
Sup. Pisa CI. Sci. 27, 1-10.
Schechter, M. (1977): Modern Methods in Partial Differential Equations. McGraw-Hili,
New York.
Schechter, M. and Snow, M. (1978): Solution of the nonlinear problem Au = Nu in a
Banach .pace. Trans. Amer. Math. Soc. 241, 69- 78.
Schechter, M. (1981): Operator Methods in Quantum Mechanics. North-Holland,
Amsterdam.
Schechter, M. (1986): Derivatives of Inappings ,vith applications to nonlinear differential
equatio'Js. Trans. Amer. Math. Soc. 293, 53 - 69.
Scheidt, J. v. and Purkert, W. (1983): Random Eigent 7 alue Problems. Akademie- Verlag,
Berlin.
Scheurle. J. (1977): Ein selekrive.-' Projektions-Iteration!iverfahren und A'iwendung auf
VerzYt'eigungsprobleme. Numer. Math. 19, 11-35.
Schmidt, E. (1907a): Entwicklung willkurlicher Funktionen nach Systemen vorgeschrie-
bener ""'unktionen. Math. Ann. 63, 433-476.
Schmidt, E. (1907b): Auj10sung der allgemeinen linearen I ntegralgleichung. Math. Ann.
64, 161-174.
Schmidt, E. (1908): Zur rfheorie der lilJearen und "ichtlinearell I luegrulgleichunyell.
Math. Ann. 65,370-399.
Schoen, R. and Yau, S. (1979): On the proof of the positive maso conjecture in getleral
relat;vit}'. Comm. Math. Phys. 65,45-76; 79,231-260.
Schoen, R. (1984): C.onformal drorlnario'l of a Riemann;a'i metric to a (O()nstalJt scalar
curvature. J. Differential Geom. 10, 479 - 495.
Schoen. R. (1986): Rece,u progress in geometric partial differential equations. In:
Berkeley (1986), pp. 121 - 135.
Schoen, R. (1988): l"'he existence of 'eak solutions with prescribed singular behavior for
a (.onformally inl'ariant ocalar equation. Comm. Pure Appl. Math. 41, 317 - 392.
Schumann, R. and Zeidler, E. (1979): The finite differen(Oe method for quasilinear
elliptic equations of order 2m. Numer. Funct. Anal. Optimize I, 161 -194.
Schumann, R. (1987): The convergence of Rothe"s method for parabolic differential
equations. Z. Anal. Anwendungen 6, 559-574.
Schumann, R. (1988): Eine Ileue Methode zur Gewinnung starker Regularitatsaussage'J
fUr das Signoriniproblem in der linearen Elastizitiitstheorie. Dissertation B, Karl-
Marx- Universitat, Leipzig.
Schumann, R. (1989): Regularity Jar Signorini's problem in elasticity. Manuscripta
Math. 63, 255-291; 455-468.
Schwartz, L. (1950): 1"heor;e des distributions, V ols. I, 2. Hermann, Paris.
Schwartz, L. (1973): Radon Measures on Arbitrary Topolog;(:al Spaces and Cylindrical
Measures. University Press, Oxford.

Referenl'CS
1155
Serrin. J (1964): Local hehal ior of so/ut;olls of quasi-linear equlltions. Acta Math. III.
247-302.
Serrin. J  1965): Isolated ,ingultlr;t;t's 0.( solutions (f qUtJs;-litlear equations. Acta Math.
113, 2 19 - 240.
Sewcll. G. (1985): Anal.r.\; of a I:illite Elel1Jent h-IerhoJ: PDE 'PROI'RAN. Springer-
Verlag. New York.
Shampine, L. and Gordon. M. (1975): Conlputer Solution. of Ordi'lary Differential
Eqlldtions. F'reeman. San Francisco. ("A
Shampine, L. and Gear. C. (J 979): A user's rie"' of solvi'lg stiff ordinary diftere,u ial
equations. 51 A M Rev. 21. 1 - 17.
Shatah. J. (1982): Glohal existence (( snulil solut iOl's to nonlinear eroillt ion eqrlations. J.
Diffcrcntial Equations 46. 409 425.
Shibata, Y. and Tsumutsi. Y. (1986): 011 a glohal existence theorem of small a'1,plit,ule
solutio"S for lIolllitlear "'are equations in an e'(terior donl"i'l. Math. Z. 191. 165 -
199.
Shokin, Ju. (1983). AfetJrvd (( Differential Approx;nlatio1l. Springer- Verlag. New York.
Showalter. R. (1977): Hilhert Spare 1\lethods (or Partial Differential Equations. Pitman,
London.
Sho\\'altcr. W. (1978): J\lecluJllics o.f Non-Ne\\'tn'l;a'l Fluids. Pergamon, Oxford.
Shubin. M. (1986J: PseudodiDere"tial Operators and Spectral Theor,r. Sprlnger- Verlag.
Nc\\' York.
Simader. C (1972): On Dir;chlefs Bou'ldary Value Prohlenl. Lecture Notes in Mathe-
matics. Vol. 268. Spranger- Verlag, Berlin.
Simader. C. (1976): ('her schK'ache IJosllrlgeu des Diriclrletprohlenls .fiir stre'IY 'I;('h,-
Iilleure ('I/ipt ische Different ialgl£';chllngen. Mat h. Z. ISO, I 26.
Simon. L. (1983): Lectures on Geolnetric Measure Theory. University of Canberra,
Australia.
Skrypnlk. I. (1973): Nonlinear Elliptic Eqllatio"s ol Higher Ordt'r. Naukova Dumka.
Klcv (R ussian).
Sk rypnik. I. (1986): Nonlinear EI/ipt if Boundary 'aille Problems. Teubner. LClplig.
Smale. S. (1965): An infinite-dimensional rersion of Sard's theorem. Amer. J. Math. 87,
861 866.
Smale, S. (1985): Efficiency of algorithnls (( a'la/rs;s Bull. Amer. Math. Sac (N.S ) 13.
94 - 118.
5mlrnov. W. (1956): IJehrgang der hohere'J .lathenlat;k, Vois. I -5. Veri der Wiss.
Berlin. (English edition: A Course in Higher Mathematics. Addison-Wesley,
Reading, M A.)
Smith. G. (1965): Nunlerical Solution.4i 0.( Partial Differential Equation$. University
Press, Oxford.
Smoller, J. (1983): Shock ",ave. a'id Reaction-Dljfu.;OI1 Equations. Spranger-Verlag.
New York.
muljan. V. (1940): (}her lineare topologische Raume. Mat. Sbornik 7 (49),425- 448.
Sobolcv. S. (1936): Methode nouvelle a resoudre Ie probleme de ('oauch.r pour les equatitJ1ls
I;II(Jires hyperbuliques nurlnale..... Mat. Sbornik I, 39 72.
Sobolev. S. (1937): 0" a boundary ,'alue proh/enl .(or the po/)'harmollic equation. Mat.
Sbornlk 2.467 -500 (Russian).
Sobolev. S. (193M): ()II a theorenl i'l !unctio'lal {lllalysi.. Mat. Sbornik 4.471 497
(R ussian).

1156
References
Soholev, S. (1950): Applications of f'utlct;onal Analysis to Mathematical Phy...ics.
lJ niversity Press, Leningrad (R ussian). (English edition: Amer. Math. Soc.,
Providence, RI, 1963.)
Sobolevskii, P. and Tiuncik, M. (1970): The finite difference nrethod for the approximate
solutioll oj. quasilinear elliptic and parabolic equations. Trudy Mat. Fak. V oronez.
lJniv. 2, 82-106 (Russian).
Sommerfeld. A. (1900): Randwertaufgahen in der Theorie der partiellen Differential-
yleichungen. In: Encyklopiidie der AI at hemat ischen Wissenschaften ( 1 899ff); Vol. 2,
Part 1.1, pp. 504 - 570.
Stackel, P. [cd.] (1894): Abhtlndillngen uher Variatio1Jsrechnung von Jollann und Jacob
Bernoulli, Euler, Lagrange, Legendre, und Jacobi. In: Ostwald's Klassiker der
exakten Wissenschaften. V ols. 46/47. Engelmann, Leipzig.
Stein. E. (1970): Singular Integrals a'id Differentiability Properties of Functioll.';. Univer..
sity Press, Princeton, NJ.
Stetter, H. (1973): A'1alysis of Discretization Methods for Ordinary Differential Equa-
t iOIl.". Springer- Verlag, Berlin.
Stoer, J. and Bulirsch, R. (1976). Einjuhrullg in die "unlerische Mathematik, Vols. 1,2.
Springer.. Verlag, Berlin. (English edition: Introduction to Numerical Analysis.
Springer.. Verlag, New York, 1980.)
Stone, M. (1932): On olle-parameter unitar}' groups in Hilbert space. Ann. of Math. 33,
643 - 648.
Stone, M. (1932a): Lillear 7'ransfornlations in ili/bert Space. Amer. Math. Soc.,
Providence. RI.
Stone. M. (1970): Remark... oj' Professor Stone. In: Browder, F. [ed.] (1970b),
pp. 236- 241.
Strang, G. and "'ix, G. (1973): All Analysis of the Fi1lite Eletnent Method. Prentice..Hall,
Englewood Cliffs, NJ.
Strauss, W. (1969): 7'he E'lergy Method i'l Nonlinear Partial Differeluial Equations.
IMP A, Rio de Janeiro.
Strocchi, F'. et al. [eds.] (1981): Topics in f"unct;onal Analysis. Scuola Norm. Sup., Pisa.
Strocchi, F. (198Ia): Classification of solutions 0.( nonlinear hyperbolic equations and
'IO'llinetlr elliptic equatio'is. In: Strocchi, F. [ed.] (1981), pp. 1-36.
Struwe, M. (1985): On the el'olut;on of harmonic mappings of Riemallniall surfaces.
Comm. Math. Helv. 60. 558 - 581.
Struwe, M. and Vivaldi, M. (1985a): Otlthe Holder co,uinuity of bounded weak solutions
of qua.'iilinear parabo/i(' i'lequalities. Ann. Mat. Pura Appl. 139, 175 - 189.
Struwe, M. (1986): NO'luniqueness i,lthe plateau problem for surfaces of consta,u mean
curvature. Arch. Rational Mech. Anal. 93, 135- 157.
Sttiben. K. and Trottenberg, U. (1982): Multigrid methods. In: l-fackbusch, W. and
Trottenbcrg, U . [cds.] (1982), pp. 1- 176.
Stummcl, F. (1970): Diskrete Konvergenz Iinearer Operatoren, I. II. Math. Ann 190,
45 - 92; Math. Z. 120, 231 - 264.
Svarc, A. (1964): The homotopic topology of Banach spaces. Dokl. Akad. Nauk SSSR
154, 61 -63 (Russian).
Synge. J. (1957): Tire H)'percircle ;n Mathematical Physi,'s. University Press, Cam-
bridge. England.
Tanabe, H. (1979): f:quutions of Evolution. Pitman, London.
Tanaka. M. (1986): Boundary Ele,ne"ts. North..lfolland. Amsterdam.

References
1157
Taniuti, T. and Nishihara, K. ( 1983): Nonlinear Wal'es. Pitman, London.
Tartar. L. (1972): Interpolation "on Ji1leaire et regularite. J. Funct. Anal. 9'1 469 489.
Tartar, L. (1983): Compacite par compensation: resultats et perspectives. Res. Notes
Math. 84, 350- 369.
Tartar, L. (1986): Ocsi/lations in Nonlinear PDEs: ConJpensated Compactness and
Iioniogeni:ation. Lectures In Applied Mathematics, Vol. 23, Amer. Math. Soc.,
Providence, RI, pp. 243 - 266.
Taubes, C. (1982): The existence of a non-minimal solution to the SU(2) Yang- .\.lills-
Iliggs equtJtio1ls on R J . Comma Math. Phys. 86, 257-320.
Taubes, C. (1982a): Self-dual Yang - A" ills connectio1ls on non-self-dual 4-maniJl}lds. J.
()ifferential Geom. 17, 139 - 170.
Taubes, C. (1984): Se(r-dual COlltJections on 4-manifolds \\'ith indefinite intersection
"Jatri.'\:. J. Differellt iat Geom. 19, 517 - 560.
Taubes, C. (1986): Physical alld mathematical applications of gauge theories. Notices of
the American Mathematical Society 33, 707 - 715.
Taylor, M. (1981): Pseudodifferential Operators. University Press, Princeton. NJ.
Taylor, C. et al. [eds.] (1987): Numericcd Methods for N01llinear Problems. Pineridge
Press, S\\'ansea, England.
Teller. E. [ed.] (1981): Fusio1l, V ols. I, 2. Academic, New York.
Temam, R. (1968): Sur la stahilite et lel convergence de la method de$ pas fractionnaires.
Ann. Mat. Pura Appl. 79. 191- 379.
Temam, R. (1970): Anal}'se nutnerique. lJniversite de -rance, Paris. (English edition:
Reidel. Boston, M A, 1973.)
Temam, R. (1977): N al'ier Stoke... Equatiolls: Theory and N umer;cal Analysis. North-
Holland, Amsterdam (Third revised edition, 1984.)
Temam, R. (1983): lVallier Stokes Equations and Nonlinear Functional A1Ialysis. SIAM,
Philadelphia, P A.
"remam, R (1986): II1!inite-dimt'nsional dynanJical syste,n$ in fluid dJllamic.. In:
Browder, I:". [cd.] (1986), Part 2, pp. 431 -445.
Temam, R. (1988): I,I/inite-Dimellsional Dynamical Systems in Alechanics and Ph)'sic....
Springer- Verlag, New York.
Thirnng, W. (1983): A Course i1l Mathenlatical Physics, Vols. I -4. Springer- Verlag,
Wien.
Thomee, V. (1984): Galerkin Finite Element Methods for Parabolic Problems. Lecture
Notes in M athematics, Vol. 1054. Springer- Verlag, Berlin.
Thompson, D. (1917: On GroK'lh and fOor,n. University Press, Cambridge, England.
Thorin, G. (1939): An extension of a cont'exity theorem due to M. Riesz. Kungl. Comma
sern. Lund 4, 1- 5.
T oda, M. ( 1981): Theory o.f N (",linear l.,att ices. Springer- Verlag, New York.
Tomi, f-". and Tromba, A. (1988): Existence Theorems for Minimal Surfaf'es of Non-Zero
Genus Spanning a Contour. Mem. Amer. Math. Soc.. Providence, RI.
Tonelli, L (1921): F ondament i di calcolo delle l'ariazioni. V ols. I. 2. Bologna.
Tornig, W., l..ipser, M. and Kaspar, B. (1985): Nutnerische Ilosung partieller Differential-
qleichungen. Teubner, Stuttgart.
Traub, J. and Wozniako\\'ski, H. (1980): A General Theory of Optimal Algorithms.
Academic, New York.
TrcfTtl, E. (1927: Ein Gege'lstilck :unJ Rilzschen Verfahren Verhandlungen des II.
Kongresses fur technische Mechanik, Zurich, pp. 131 - 138.

1158
References
TrefTtl E. (1928): KO,llJeryenz und f'ehlerabschiitzung beim Ritzsche'l Verfahren. Math.
Ann. IOO 503 - 521.
Treves, F. (1975): Basic Linear Partial Differential Operators. Academic Press, New
York.
Treves, F. (1982): Introduction to Pseudodifferential and f()urier 1,1legral Operators.
Plenum, New York.
Triebel, H. (1972): Ilijhere A'ialysis. Veri. der Wiss., Berlin.
Triebel, H. (1913): Spa('es of distributions of Besov lype on Euclidean n-space. DuaUt.v,
interpolatioll. Arkiv Mat. II, 13-64.
Triebel, H. (1978): Interpolation Theory, FU'lct;on Spaces, Differential Operators. VerI.
der Wiss., Berlin.
Tnebel, H. (1981): Analysis und mallaematische Physik. Teubner, Leipzig. (English
edition: Teubner, Leipzig, 1983.)
Triebcl, H. (19M3): 1"laeury of f"unClio1l Spaces. Geest & Portig, Leiplig.
Tromba, A. [ed.] (1987): Set,.inar on Ne' Results itl Nonlitlear Partial Differe1ltial
Equatiol1s. Vieweg, Braunschweig.
Troyanski, S. (1971): Otl locally unifornlly convex and differentiable norms in certain
1Iotl-separable spaces. Studia Math. 37, 173-180.
Turner, R. (1974): Positive solutions of nonlitlear e;ge"value problem..... In: Prodi, G. [cd.],
Eigetlvalues of N unUnear Problems, pp. 213- 239, Ed. Cremonese, Roma.
Tychonov, A. and Samarskii, A. (1959): Differe,uialgleichungen der Mathemat i!tchen
Ph}'sik. VerI. der Wiss., Berlin. (English edition: Partial Differenlial Equations of
Mathe"lat;cal PIrJ'sics, Holden Day, New York, 1964.)
Uhlenbeck, K. (1982): ContJectiolis ';lh Lp-bounds on curvature. ('omm. Math. Phys.
83.31-42.
Ulam, S. (1976): Advetuures of a Iathematician. Scribners. New York.
Uralceva, N. (1987): Otl tire regular;ly oJ the solutions oj' variational inequalities.
Uspekhi Mat. Nauk 42 (6), 151 -174.
Vahanija, N. el al. (1985): Ratldot,. Distributions in Banach Spaces. Nauka, Moscow
(R ussian).
Vainberg, M. (1956): Variational Methods for the Stud}' of Nonlinear Operators.
Gostehizdat, Moscow (Russian). (English edition: Holden Day, San f"rancisco,
C A, 1964.)
Vainberg, M. (1972): 1"he Variational Method and the Method of Monotone Operators.
Nauka, Moscow (Russian). (English edition: MonotcJlle Operators, Wiley, New
York, 1973.)
Vainikko, G. (1976): FUllkt;otzalunal}'sis der DiskretisierulIgsverfahren. Teubner.
Lei pzig.
Vainikko, G. (1977): 0 ber die K ont'eryenz utJd Di(,ergenz von N iiherungsmethodetl he;
Eigenwertproblemen. Math. Nachr. 78, 145-164.
Vainikko, G. (1978): Approx;matil'e tnethods jor nota/itrear equclliotls. Nonlinear
Anal. 2, 647 - 687.
Vainikko, G. (1979): Regular convergence of operators and the approximate solution of
equations. Itogi nauki i tehniki, Mat. Anal. 16, 5-53 (Russian).
Valent, T. (1988): Boundary Value Problems of f";nite Elastic.ity. Springer- Verlag, New
York.

Rcferenl.'Cs
1159
Valentine, (-... (1945): A I..ipsc"iIZ cO,ldil ion preserl'ing extension for tJ l'ector .tunct ion.
Amer. J. Math. 67. 83 93.
Varga. R. (1962): A'atrix Iterative Analysis. Prentice-Hall, Englewood Cliffs. NJ.
Varga, R. (1971): Functional Analysis and Approximation TIJeor)' i'l Nunlerica/ .4nal.\'sis.
SIAM. Philadelphia, PA.
Varga, R. (1984): .4 ...urrey of reee"t results on iteratil'e nJethods lor sol,'ing large sparse
lillear s,\'stems. In: Birkhoff, G. and Schoenstadt, A. [eds.] (1984), pp. 197 -217.
Vejvoda, O. et al. (1981): Partial Differe,Jlial Equatio1ls. Noordhoff, Groningen.
Velte, W. (1976): Direkte Methoden der Variationsrechnuny. Teubner, Stuttgart.
Vel tee W. (J 984): Bounds for cri t ;cal val14fs and e;genfrequencies of mechanical s .\'st ems.
In: 80m. V. and Neunzert. H. [eds.] (1984), pp. 469-484.
Vilcnkin. N. (1975): Cf. Vlrcenko. N. [ed.] (1983). p. 228.
Vinogradov. A.. Krasilcik, I.. and Lycagin, V. (1986): 111troduction to the Geometry (r
Nonlinear Partial Dfferential Equations. Nauka, Moscow (Russian).
Virecnko, N. [cd.] (1983): Mathematics: A Collection r Quotations. Vysca Skola. Kiev
(R ussian).
Visik. M. (1949): The method of orthogonal projection and direct decomposition in the
theory of d(ffre'lIitd equations. Mat. Sbornik 25. 189 234 (Russian).
Visik. M (1951): On ..;tro,ayl.r elliptic differential equations. Mat Sbornik 29. 615 -676
( Russian).
Visik. M, (1952): On general bOllndar}' I'alfle prohlem.... _for elliptic differe,"ial equations.
Trudy Moskov. Mat. Obscestva I. 187-246 (Russian).
Visik. M. (1961): Bou1ldary value prohle,ns for quasi/inear strongly elliptic eqllat ions.
Dokl. Akad. Nauk SSSR 138,518-521 (Russian).
V isik. M. ( 1962): On in;1 ial houndary l'alue problems for quasi/inear parabolic equal ions
o.rlJigher urder. Mat. Sbornik 59, 289- 325 (Russian).
Visik, M. () 963): Quasi/inear strongly elliptic systems of diDerelltial equations in
dil'ergeJlce ic)rn.. Trudy Moskov. Mat. Obscestva 12. 125-184 (Russian).
Visik. M. and Fursikov. A. (1981): Mathematical Problems in Statistical Hydro-
mechanics. Nauka. Moscow (Russian). (German edition: Mathenlatische Prohleme
der stalistischen JI.\'dromecha1Jik. Teubner. Leipzig, 1986.)
Visik, M. and K uksin. S. (1986): Perturhation o..f quasi/inear elliptic equations and
Fredholm t1ltl'lifulds. Mat. Sbornik 130, 222 -- 242 (R ussian).
Vladimirov. V. (1971): Equations o.f Mathenlatical Physics. Dekker, New York.
Vladimirov. V. [ed.] (1986): Collection o.l Surl'ey Articles on the Occasion oj the 50th
Annil'ersary of the Steklul' Institute. Trudy Mat. Inst. Steklov. 175 (Russian).
Vladimirov, V. [ed.] (1986a): ,4 Collection of Prohlems 011 the Equations of .alht-
",atical Physics. Mir. Moscow (English edition).
Wahl, W. v. (1978): ('ber die stat;onaren Gleichungen l'on Navier- Stokes. semilineare
el/iptische uIJd paraholische Gle;chungen. Jahresber. Deutsch. Math.- Verein. SO,
129 149.
Wahl. W. v. (1978a): Die stat;onaren Gleichungen I'on NU1';er-Stokes und semi/ineare
el/iptische Systeme. Amer. J. Math. 100, 1173 1184.
Wahl. w. v. (1982): Nichtlineare EI'olotionsgleichungen. In: Kurke, H. el al. [eds.],
Rt'cent 1"rends in Mathematics. pp. 294 -302. Teubner, Leipzig.
Wahl, W. v. (1982a): 1'he equation u' + A(t)u = f in Hilhert space and l.- p -estimates for
parabolic eqllatio"s. J. London Math. Soc. (2), 25.483 - 497.

1160
References
Wahl, W. v. (1985): The EquutiolJS of N luier-St()kes and Abstract Parabolic Eqllations.
V ieweg, Bra u nsch wcig.
Walter, W. (1964): Differential- und Illtegralungleichungen. Springer- Verlag, Berlin.
Walter. W. (1985): Functional differential equations of tile Cauchy- KOl'alel'skaja t}'pe.
Aequationes Math. 28. 102-103.
Warsaw (1983): Proceedillgs of the Int('rnat;onul C()n/reSs oj' MathematicialJs ill
Warsa"', Vols. 1,2. PWN, Warsaw.
Wegert. E. (1987): 7opolog;cal methods for strongly nOlllinear Riemann- Hilbert prob-
leIPJs for lJ%lnorpluc /illictions. Math. Nachr. 134, 201 230.
Weierstrass, K. (1870): 0 ber dtls sogenatlnte Dir;chlelsche Prinz;p, In: Weierstrass, K.
( 1894), Vol. 2, pp. 49- 54.
Weierstrass, K. (1894): Mathelnatisclze 'erke (Collected Works), Vols. I -7. Berlin,
1894 1927.
Wcinbcr, S. (1986): Cf Altlthelnat;c.'t: 7"he Uni}ying "Thread ill Sciellce (1986).
Weinberger, H. (1974): Var;at;ollal Methods for Eigenvalue Approximatiol1. SIAM,
Philadelphia, PA.
Weinstein, A. and Stenger. W. (1972): Methods of Intern.ediate Problems for Eigen-
l'alues. Academic Press, New York.
Wells, R. [ed.] (1988): The Alatliematicailieritage of Ilermatln Weyl. Amer. Math. Soc.,
Pro\'idence, R I.
Wendland, W. (1979): Elliptic Systems ila the Plane. Pitman, London.
Wendland, W. ( 1984): OIl ..,ome mathenllll;cal aspects of boundary elemelu Inethods ./flr
elliptic problems. In: Whiteman, J. [ed.], 7'he lathen'(ltics oj' f';nite Elelnents alld
Applicatiolls. pp. 193 227. Academic Press, New York.
Wente. H. (1986): ('ounterexample to tl fOlljecture of Heinz Ilopf. Pacific J. Math. 121,
193-243.
Weyer, J. { 1982): M a,inlal Inolloton;c;t.r of operators wit h sffic;elJlly large domaill tlnd
upplicatiolls to the Hartree problem. Manuscripta Math. 38, 163-174.
Weyl, H. (1911): 0 ber die asy"'ptotische VerteiluIlg der Eige,nverte. Gottinger Nachr.
pp. 110 117.
Weyl, H. (1940): The "Iethod of orthogonal projectioll in potential theory. Duke Math.
J. 7, 411 . 444.
Weyl, H. (1944): Datid Ili/berl and his InatlJelnat;cal work. Bull. Amer. Math. Soc. SO,
612 - 654.
Weyl, H. (1968): Collected Works. Vols. 1--4. Springer-Verlag. New York.
Whitham, G. (1974): l.jllear and N onlil.ear Waves. Wiley, New York.
Widder, D. (1975): The Heat Equatioll. Academic Press, New York.
Wille, F. (1972): Galerkins I-JosulJgsanniiherungen he; monotonen Abhildungen. Math. Z.
127, 1 0 - 16 (A poeln).
Wilkinson, J. (1965): 7'lIe Algehra;c Eiyelll'alue Problem. Clarendon, Oxford.
Wi lIough by. R. [ed.] ( 1974): St iff DfJerelu ial S }'...t ems. Plen urn, New York.
Wilson, K. (1982): The rellornzalizatioll group ulld critical phenomena. In: Nobel PrIze
IJectllres, Vol. 1982, pp. 57-87, Stockholm.
Witten, E. (1986): Ph}'s;c.-. alJd Geolnetry. I n: Berkeley (1986), pp. 267- 306.
Wloka, J. (1982): Partielle DijJerentialgleiL'hungen. Teubner, Stuttgart. (English edition:
Purt;ul D;lTerelltial Equations, University Press, Cambridge, England, 1987.)
Wolfersdorf, L. v. (1982): Monotonic;t}' nJethods .for two classes of nonlinear hOlll.dar}'
('a/ue problen.s ,,'ith sel'li!illear first-order syste".s in tile pilule. Math. Nachr. 109,
215 238.

References
1161
Wolfersdorf. L. v. (1983): MOllotollicity nrethotls jl)r IIonlinear singular integro-
differt'luial equations. Z. Angew. Math. Mech. 63,249-259.
W olfersdorf. L. v. (1987): A class of nonlinear Riemann -II ilhert problems K;t h monotone
non/inecJrities. Math. Nachr. 130.. 111 119.
Wollman, S (1984): An existence and uniqut'ness tlJeore'" for tlJe rlasol' - Ma.'(K,(,J/
...ystem. Lomm. Pure Appl. Math. 37.457 -477.
Wusslng, H. and Arnold, W. (1975): Biograph;("1 hedeutellder ,\1athematiker. Volk und
Wlssen. Berlin
Ya u, s. ( 1978): 0" the R icei curr'ilt ure of a compact K iihler '"anrold and the complex
J\lo1lye Alnpere equation. Comm. Pure Appl. Math. 31, 339 -411.
Yau, S. (1986): Nonlinear Analysis In Geo"Jetry. L'Enseignement Mathematique.
Geneva:
Yoslda, K. (1948): 0" the diflerenliahi/ity a'id tile representCJtion (r o'Je-paranleter
s(Jmigrvups or lirrear operators. J. Math. Soc. Japan I, 15 21.
Yosida, K. (1965): Functional Analysis. Springer- Verlag, Berlin.
Zabreiko, P. and Krasnoselskii. M. (1975): Inlegrcli Equations. Noordhoff. Leyden.
Zarantonello. E (1960): SolrillY .runct iOllal equat ions hy contract i,'(' al'eragin M athe-
malies Research Center Rep. # 160, Madison, WI.
Zarantonello. E. (1964): The closure of the 'Jllmerical range contains tile specrrunJ. Bull.
Amer. Math. Soc. 70, 781 - 787.
Za ran tonello, E. [ed ] (1971): C ont r;"111 ions I () N ollli"ear A nalysis. Academic Press.
New York.
Zarantonello, E. (1973): Delise sinyle-l'aluedness of monotone operators. Israel J. Math.
15, 158 166.
Zaranlonello. E. (1981): CO'liCLlI spectral theory. In: Strocchi. F. [cd.] (1981). pp.
37 116.
Zeidler, E. (1980): 1'he Ljusternik - SchirelnJan theory for iIJdefinite and not necessarily
odd 1JolJ/"Jear operLltors arid its applications. Nonlinear Anal. 4,451 -489.
ZCldler. E. (1986): LJusternik - Sch'lirelman theor" OIl generallerel sets. Math. Nachr.
129, 235 - 259.
Ziamal, M. (1968): On the (i'lile elenrent method. Numer. Math. 12.394--409.
Zurmiihl. R. (1965): Praktische Mathematik. Springer-Verlag, Berlin (Fifth edition
1984 ).


List of Symbols
We use the following abbreviations:
B-space Banach space
H-space Hilbert space
M S sequence Moore-Smith sequence
F-derivative Frechet derivative
G-derivative Gateaux derivative
AI(JO) means (10) in the Appendix to Part i
General Notation
n,u, -
.91 implies fM
if and only if
r4 iff &f
f(x) = 2x by definition
x is an element of the set S
x is not an element of S
set of all x with the property. . .
the set S is contained in the set T
S is properly contained in T
identical to S eT, where S denotes the closure
ofS
intersection, union, difference
empty set
set of all subsets of the set S, the power set
ofS
. :=. 
iff
J1/
f(X) d 2x
xeS
xtjS
{x: . . . }
S C T
Sc T
See T
o
2 5
1163

1164
XxY
N
R.C,O,l
IK
R.
R>
R'"
R
Rez,lmz
[a.b),]a,b[.[a,b[
I, id
f: S C X -t> Y
D(f)
R(/)
N(f)
G(f)
domf, iml
kerf
f surjective
I injective
f bijective
I(A)
f -1 (B)
IL.
fog
f: S -+ 2 T
R(f)
G(/)
dom J: D(f)
'J
g(x) = o(f(x», . -. a
g(x) = O(f(x», x .-. a
B'
SN
sgna
List of Symbols
product set, X x Y = {(x,},): x e X.y e Y}
set of the natural numbers 1, 2, · . ·
set of real, complex, rational, integer numbers
RorC
set of nonnegative real numbers   0
set of positive real numbers  > 0
set of all real N-tuples x = (1'" ., .'l)
set of all x e R N with i  0 for all i
real part of the complex number z. imaginary
part of z
closed, open, half-open real interval
identity mapping
mapping from the set S into the set Y with
S c X
domain of f, D(f) = S
range of I, R(f) = f(S)
null space of f, N(J') = {x: f(x) = O}
graph of f, G(f) = {(x,f(x): . e S}
identical to D(f R(f)
identical to N(f)
mapping onto Y, i.e.. /(5) = y
one-to-one mapping
one-to-one mapping onto Y, i.e., f is surjective
and injective
image of the set A, f{A) = {f(x): x e A}
preimage of the set B, 1- 1 (B) = {x:f(x) E B}
restriction of the map f to the set A
f applied to g, (f 0 g)(..) = f(g(x»
multivalued mapping, I(x) is a subset of T
range of the multivalued mapping J: R(f) =
UJCEsf(x)
graph of the multivalued mapping J: G(f) =
{ (x, }'): XES,)' E I( x) }
the effective domain of the multivalued map-
ping J: doml = {x: f(x) :F 0}
Kronecker symbol, ij = 1 if i = j and b 'j = 0
if i :f:. j
g(x)/!(x)  0 as x --. a
Ig(x)1 S const I/(x)1 for aU x in a neighborhood
of the point a
closed unit ball in R h ', B. tt = {x e R N : I..I < I}
N-dimensional unit sphere, S'v = {x e RJ\':
Ixl = I}
signum of the real number a

Special Notation
1165
Special Notation
In persuing the following symbols, the reader should pay attention to the
possible danger of confusion.
The page numbers 1 10 and 10 refer to page 10 of Part I and the present Part
II, respectively.
Page
X. dual space to X 1774
A* dual operator to A in a B-space, transposed matrix 1775
A.. adjoint operator to A in an H-space, adjoint matrix
(transposed and conjugate complex) 1786, 124
Observe that if the continuous linear operator A: X -t X is defined on the
H-space X, then the operators A*: X. -+ X* and A..: X -+ X are defined on
dfferent spaces.
F'
F-derivative or G-dcrivativc of the operator F
(the text always refers precisely to the momentary
meaning) 1135
partial F-derivativc or G-derivative of F with re-
spect to the variable x (the text al\\'a}'s refers pre-
cisely to the momentary meaning) 1140
inner (scalar) product on an H-space 7
ordered pair, an element from the product set X x Y
inner (scalar) product in RA' or C A ' 1771
value of the linear functional f at the point x,/(x)
convergence in norm 1769
weak convergence 255
weak- convergence of functionals 260
uniform convergence of functions 1801
another notation for the mapping I
another notation for the mapping f
subsequence of (XII)
norm of x in a B-space 1768
Euclidean norm of x, Ix I = (XIX)112
p-norm of the point x in R'v or norm of the function
f in the Lebesgue space Lp(G) (the text always refers
precisely to the momentary meaning) 1771. 35, 36
F-difTerential (Frechet differential) of the mapping
F at the point u in direction h 1 135
nth F-differential of F at the point u in the directions
hI' · · · , hIt 1143
identical to d" F(u; h,. . . ,h)
first variation of the mapping F at the point u in
direction h 1143, 689
F;x
(:( I}')
(x, y)
(xly)
(.f, x)
XII -. X
XIIX
f,,f
f,,f
x  f(,,)
f(. )
(XII. )
Ii  II
Ixl
IIxli p . 111111'
df'(u; h)
d"F(u; h J .. · . t hIt)
dIlF(u; h)
f'(u: h)

1166
"f.(u; h"..., h,,}
c}"F(u; h)
fO' (u)h
f'''(u)hk
f."(u)h 2
List of Symbols
nth variation of f' at the point u in the directions
hi' . . . , hIt
nth variation of F at the point u in direction h
(identical to C>"F(u; h,. . . ,h))
F'(u) applied to h
identical to (F"(u)h}k
identical to F"(u)hh
1143
689
1135
1136, 1144
Note the following. The nth F -derivative F(II)(u) exists at the point u iff the nth
F-difTerential d"f.(u;...) exists at the point u. In this case, we have
F(") ( u) hi' . . II" = d" F ( u; hi' . . . , h,,) = b" F ( u; hi' . . . , h,,)
for all hi' . . . , hIt. In particular, we get
f'(11)(I4)h" = d" F(u; h} = C)" F(u; h)
for all h (see page 144 of Part I).
General Notation Introduced in Part I
(1S
S
int S
U(x)
U(x, R)
-
U (x, R)
supp .r
tim , lim
diam S, d(S)
dist(x, S), d(x, S)
distx(x, S)
dist(S, T), d(S, T)
S + T
)S
span S
coS
coS
dimL
codim L
x/>r
X(f)Y
boundary of the set S
closure of S
interior of S
neighborhood of the point x
open ball with center x and radius R, U(x, R) =
{Y:lly-xll <R}
closed ball, U(x,R) = {y: lIy - xii < R}
support of the function (distribution) f
lower, upper limit
diameter of the set S
distance of the point x from the set S
distance of x from S in the space X
distance between the sets Sand T 1762
sum of the sets Sand T 1764
product of the set S by the number A. 1764
linear hull of S 1764
convex hull of S 1764
closed convex hull of S 1764
dimension of the linear subspace L 1765
codimension of the linear subspace L 1765
factor space 1765, 1770
direct sum or topological direct sum (the text always
refers precisely to the momentary meaning) 1765, 1766
1751
1751
1751
1751
1756, 1048
1761
1762
1762

Function Spaces Introduced in Part I
1167
Xl
complement of X with respect to a direct sum or
orthogonal complement to X (the text always refers
precisely to the momentary meaning) 1766, 65
product space 1755, 1770
general product space 1755
complexification of the real B-space X 1770
complexification of the linear operator A 1770
rank of the linear operator A, rank A = dim R(A)
index of the linear operator A, ind A = dim N(A) -
codim R(A)
index of the nonlinear Fredholm operator F 667
determinant of the linear operator A 1179
resolvent set of the linear operator A 1795
spectrum of the linear operator A 1795
spectral radius of the linear operator A 1795
XxY
n X"
X(
A(
rank A
ind A
i nd f
detA
p(A)
utA)
r(A)
In rea) B-spaces, p(A), u(A), and r(A) always refer to the complexification Ac,
i.e., 1)(A) = p(A(), etc. 1770
Function Spaces Introduced in Part I
In the following, G denotes a nonempty bounded open set in IR N , N > I. Let
- oc) < a < h < 00. Moreover, let k = 0, I,... and 0 <   I.
('G E Cle. (J
boundary property of the set G (If k > I, then the
boundary oG of G is smooth.)
piecewise smooth boundary
special decomposition of oG
space of linear continuous operators from X into
y
space of continuous operators from X into Y
space of k-times continuously F -differentiable func-
tions from X into Y
identical to C(X, Y)
Holder constant of u, i.e., Ha(u) is the smallest con-
stant L with
1232
1233, 18
522
i1G E Co. I
aG = i\ G u O 2 G
L(X, Y)
C(X, Y)
CIt(X, Y)
1135
1148
1148
CO(X, Y)
Ha(u)
II u II Cia. hJ
II u II Ck(a, h]
II U IICk"(a,h)
lu(x) - u(y)1 < Llx - ylCi
for all ., Y E G.
identical to sUPaxh lu(_)1
. d . I ' Ie I (j) ( I
I entlca to j=O sUPaSxb u x)
identical to lIu/lCJcla,b} ! H(J(u(Ie)), where the Holder
constant H:J refers to G = [a,b]

1168
List of Symbols
(lu U CCG )
II U II CJc(l: I
II U IIcJc'.(G)
identical to supxc l: lu(x)1
identical to LIPIs;, supxG lD'u(x)1
identical to
II u IIclc(G) + L H.(D*u).
IPI-'
Observe that flu!lccG) = lillUoo for continuous functions u: G -. R (see page
36).
C',cr[a. b]
real B-space of all continuous functions u: (a, b] -.
R with the norm 1114tlt1d.bJ
real B-space of all k-timcs continuously differentia-
ble functions 14: [a, b] -+ R with the norm l!un(Q.b]
real B-space of all functions U E C' [a, b] with
IJr41)C"'.(G.6J < 00.
The norm on CA,cr[a, b] is given by lIu IICJc.....bl:
real B-spacc of all continuous functions u: G -+ R
with the norm lIullc(G)
real B-space of all k-timcs continuously differentia-
ble functions u: G -+ R with the norm lIu lI(eJc«]).
(More precisely, Ck( G) consists of all continuous
functions u: G -. iii which are k-times continuously
differentiable on G and all of whose partial deriva-
tives up to kth order can be continuously extentcd
to the closure G of G.)
real B-space of all functions u E C'( G) with
II ullct-.e(G) < 00.
The norm on C.cr( G) is given by lIuU c Jc'8(c),
identical to C(G)
identical to C«( G) for 0 < a < I.
(.[a b]
CA[a. b]
C(G)
C'( G)
c',cr( G)
CO(G)
CO'«( G)
Observe that CO. 1 (G) denotes a space of Lipschitz continuous functions,
whereas C 1 ( G) denotes a space of continuously differentiable functions.
Cb(R " )
real B-space of all bounded continuous functions
u: (JiN -. R with the norm IluUCCR")
real B-space of all k-timcs continuously ditTerentia-
ble functions 14: RJ\' -+ R with
II u II CJc(R..) < 00.
The norm on C:(RJ\') is given by lIullck(tR).
If we speak of the B-space C( G) with G = AN, then C( G) is understood to be
Cb(R"').
C:(IRN)

Function Spaces Introduced in the Present Part II
1169
X" product space of X, i.e., X" consists of all u =
(u 1 , · · · , U"  where Ui E X for all ;
If X is a B-space over II( = iii. C. then X,. is also a B-space over IK with the norm
.
lIuli = L lIu t II.
i=1
This way, e obtain the real B-spaces C(G)", Lp(G)", W,'"(Gf, etc., and the
spaces CX'(Gr, Co(G)", etc.
Function Spaces Introduced in the Present Part II
-
CCC(G)
Co (G)
Observe that the notations Co(G \ G Lp(G) Wp"'(G), and W;'(G) are of
fundamental importance for the present Part II.
CXJ(G) space of infinitely continuously differentiable func-
tions u: G -+ R
u E C( G) iff u e ( G) for all k = 1, 2, ...
space of all functions II e COO(G) with compact sup-
port in G
identical to
17
18
lIuli p
1I1I11.x.
111111 p.06
II U II'XI.G
1I1I1I"'eP
(fG lul' d:<YIP,
\vhere 1 < P < 00
identical to ess sUPJec G lu(x)1
identical to (JiG I III' dX)l/P, where I S p < CX)
identical to ess sUPJec(tG lu(x)1
identical to
36
( L f. I D2UIPdx ) I/P;
«Im G
Ii rlllJ 11I.,.0
norm on the Sobole\' space Wpm(G), where I S P < 00
and m = 0, 1, 2, . . .
identical to
( L f. IDIUIPdX ) IIP,
«1=11I G
lIullm,a:
where 1 S P < 00 and m = 0, I.. . . .
identical to LI«IS:," IIDGrulJoo; norm on the Sobolev
space W:( G), where m = 0, I, . . .
identical to Ller):::.. HD 2 ull«.
identical to
II u II m, tL'. 0
( U It,) 2
fG ut 'dx;

1170
list of Symbols
(ul V)m. 2
scalar product on L 2 (G) and L(G). (Note that ii = u
on L 2 (G), where the bar denotes the conjugate com-
plex number.)
identical to
L f. DfJ u DtJvdx;
lal S '" G
scalar product on W 2 "'(G) and W 2 '"(G}c. (Note that
Ii = u on W 2 "'(G).)
Observe that lI'II""p and lI'II""p.o are equivalent norms on the Sobolev space
Wp'"(G), where 1  p < 00 and m = 0, 1,2, ,.,
Lp(G) Lebesgue space of all measurable functions u: G -+ R
with
/tulip < 00,
where 1 < p  00
Lebesgue space of all measurable functions u: aG -+
R with lIullp.c1G < 00, where I  p < 00, (Note that
the measurability of u refers to the surface measure
on (lG.)
Lebesgue space of all measurable functions u: G -+ K
with lIulip < 00, where I( = R, C and 1  P  00.
(Note that L(G) = Lp(G).)
Sobolev space of functions u: G  R, where m = 0,
I, . . . and I :5: P  00 236, 237
closure of C() in W,"'(G) 237
closure of COO(G) in W,'"(G) 242
Sobolev space of functions u: iJG -+ R for real m > 0 1031
special Sobolev space for functions u: iJG -+ R 530
Sobolev space offunctions u: G -+ IK for real m, where
IK = R, C
Wp'"(G)f( closure of Co(G)1( in Wp'"(G)" for m  0
Observe that Wp"'(G) = Wp'"(G)R and that
-"'(G) = Wp"'(G)* (E)
for all real m > 0, I < P < 00, and p -I + q -1 = I. Here, the asterisk denotes
the dual space. Equation (E) remains true if we replace -"'(G) and Wp"'(G)
with the corresponding complex Sobolev spaces -'"(G)c and Wp'"(G)e,
respectively.
35,36
Lp(iJG)
L(G)
Wpm(G)
Wp'"(G)
1r'"( G)
W;(aG)
W p l / 2 (iJ j G)
Wp'"(G)K
1061
H'"
H'"
c
identical to W 2 "'(R N )
identical to W2,"(N)C'
Observe that
Hm c H k
and
H '" C H t
C - C
for all real m  k.

Notation Introduced In the Present Part II
1171
In particular, we obtain that
...H 2 c HI C H O C H- ' C H- 2 ...,
where HO = L 2 (fRN).
W p o ( G)
Wpo(G)r
L p . 1oc ( G)
"Jl C H c V."
Lp(O. T; X)
W p l (0. T; V, H)
C w( [0. ex. ['-H)
B.4
Ft'4
L p. loc ( G. Y)
Lp(M -+ Y. Jl)
BV(G)
fIJ", "."., i"
sPp(,,)
o
11 'pm(,,)
'ulm.p.lulp,,,..o
!I(G.IK)
!/(G)
:J'(G. Y)
9'(G)
Y( R'V )
,'(RN)
Hm( A)
ff'"( A)
identical to Lp(G) for I < p  00
identical to L(G) for 1 < P < 00
space of all functions u: G -+ R with U E L p ( G) for all
compact subsets C of G
evolution triple
Lebesgue space of functions u: ]0, T[ -+ X
special Sobolev space of functions u: ]0, T[ --+ V with
respect to the evolution triple "V c H c V."
space of weakly continuous functions u: [0, ex [ --+ 1/
Besov space
Triebel- Lizorkin space
space of all functions u: G -+ Y with Ie lIu(x)lffdx <
oc for all compact subsets C of G
Lebesgue space of functions u: M -+ Y with respect
to the measure JJ
space of all functions u: G -+ IR of bounded variation
special sets of grid points
discrete Lebesgue space corresponding to Lp(G)
discrete Sobolev space corresponding to W p "'( G)
discrete Sobolcv norm corresponding to II u II m, 1"
Ilullm,p.o, respectively
space of infinitely continuously differentiable func-
tions 14: G -+ IK with compact support in G 1046
identical to C o '( G) 18, 1045
space of distributions with values in the B-space Y 1047
identical to '(G,IR)
space of infinitely continuously differentiable func-
tions which go rapidly to zero as Ixl -+ 00
space of tempered distributions
m-dimensional Hausdorff measure of the set A
m-dimensional normalized Hausdorff measure of the
set A
416
417
422
784
1106
1106
1047
1071
1114
986
987
987
987
1058
1060
III 1
1112
Notation Introduced in the Present Part II
x = X.
..\' .
D . = ( /)"..
. ., .
identification of the real H-space X with the
dual space X. 254
antidual space to the complex H-space X 67
partial derivative with respect to i (in the
classical or generalized sense) 61. 232

1172
 = (I'...'!XN)
'I
DClu = DI . . . D;tu
i1u/lln
N
 = L Dl
;=1
V! "
VA'
Vi' V/:
va, Vi
" = V _"V"
meas G
Jc;f dx
I(;c; f dO
JGf d
Jl(A)
J u" dx"
Sf.
A c B
{E,! }
L(f)M
(M), (S), (S)+, (S)o
(P)
ns(b)
deg( f', G y)
deg( f'", G)
i(f, G)
deg(B, M; G)
DEG(f, G, y)
List of Symbols
multi-index 231
identical to I!XII + ... + IIINI 231
partial derivative of order IC(I of the function u
(Note that DClu = u for !X = 0.) 231
outer normal derivative of the function u,
ou/(!n = L I n;D;u, where n = (n I' . · ., n N ) de-
notes the outer unit normal 20
Laplacian 19
difference operator 199
difference operator with respect to the variable I 204
difference operator with respect to the variable
i; this difference operator corresponds to the
differential operator D j
difference operator corresponding to the dif-
feren tial opera tor D fJ
special difference operator of second order
(discrete Laplacian)
Lebesgue measure of the set G
Lebesgue integral with respect to the N-dimen-
sional Lebesgue measure, where G c n N
surface integral
integral with respect to the measure Jl
measure of the set A with respect to the mea-
sure Jl
discrete integral identical to the sum Lp u,,(P)h N
smoothing operator
the operator B is an extension of the operator A
spectral family
orthogonal direct sum of the two linear sub-
spaces Land M
operator properties
operator property
number of solutions of the equation Au = b,
UES
Leray Schauder mapping degree with respect
to the equation F(x) = y, X E G (see page 531 of
Part I)
identical to deg("", G, y) for y = 0
red-point index of the compact mapping .f:
G c X --. X (Note that ;(/, G) = deg(1 - Jt G).)
coincidence degree with respect to the equation
Bx = Mx, x E G 646,737
multivalued Browder-- Petryshyn degree with
respect to the equation f.(x) = y, X E G
986
986
199
1010
1014
1023
1071
1064
987
73
124
1084
266
583
586
675
1004
999

Notation Introduced in the Present Par. II
oG: Fo  f- I (mod }')
cG: 10 "" /1
J
U.V
U@V
b"
b
-+
U -.:II. U
II
4
"It -. "
d.
u. -. II.
II
S T
S.(x)
[X, Y]'.P
[X. Y],
homotopy mod y (see page 569 of Part I)
homotopy (see page 527 of Part I)
duality map
convolution of distributions
tensor product of distributions
Dirac's delta distribution at the point x
identical to % for . = 0
weak+ convergence
discrete convergence
discrete- convergence
the set S is -,,'m os t equal to the set 1', i.e.. iot S =
int T and S = T
viscosity derivative
interpolation space (K-mcthod)
interpolation space (complex method)
1173
67,860
1051
1051
1047
784
982
982
906
950
1102
1108

List of Theorems
Mathematics is endangered b)' a loss of unity and interaction.
Richard Courant (1888-1972)
l-heorem 18.A
Theorem 18.8
Theorem 18.C
Theorem 18.0
Theorem 18.E
Theorem 18.F
Theorem 18.0
Theorem 18.1-1
Theorem 19.A
Theorem 19.B
Theorem 19.C
(Main theorem on quadratic minimum problems). . . S6
(The Dirichlet principle) . . . . . . . . . . . . . . . . . . . . . . . . 63
(The perpendicular principle and orthogonal decom-
position in Hilbert spaces). . . . . . . . . . . . . . . . . . . . . . . 65
(The R iesz theorem). . . . . . . . . . . . . . . . . . . . . . . . . . . . 66
(The Lax-Milgram lemma) . . . . . . . . . . . . . . . . . . . . . . 68
(The main theorem on linear monotone operators) . . 69
(The lemma of Weyl on the regularity of generalized
solutions for the Dirichlet problem). . . . . . . . . . . . . . . 78
(The Euler- Lagrange equation for general variational
problems) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 86
(Completeness of orthonormal systems) . . . . . . . . . . . 118
(Eigenvalues of linear symmetric compact operators-
the Hilbert-Schmidt theory. . . . . . . . . . . . . . . . . . . . . 119
(The Friedrichs extension of linear symmetric opera-
tors). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 126
Theorem 19.0 (Main theorem on abstract linear parabolic equa-
Theorem 19.E
Theorem 19.F
Theorem 19.G
tions) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 1 S3
(Monotone operators and the main theorem on linear
nonexpansive semigroups) . . . . . . . . . . . . . . . . . . . . . . 160
(Main theorem on one-parameter unitary groups). . . 160
(Main theorem on abstract semilinear hyperbolic
equations) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 166
Theorem 19.H (Main theorem on the abstract semilinear Schrodinger
1174
equation). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 168

List of Thorems
1175
Theorem 19.1 (Fractional powers of operators. abstract Sobolev
spaces, and the main theorem on abstract semilinear
para bolic eq uations) . . . . . . . . . . . . . . . . . . . . . . . . . . . 169
Theorem 19.J (Five general uniqueness principles for operator equa-
tions and monotone operators) . . . . . . . . . . . . . . . . . . 174
Theorem 19.K (A general existence principle for operator equations
and monotone operators) . . . . . . . . . . . . . . . . . . . . . . . 176
Theorem 20.A (Main theorem on the convergence of abstract dif-
ference schemes). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 196
Theorem 20.8 (The equivalence between stability and convergence
for approximate solutions of well-posed and consis-
tent linear operator equations). . . . . . . . . . . . . . . . . . . 211
Theorem 20.C (The equivalence between stability and convergence
for approximate solutions of well-posed and consis-
tent linear evolution equations) . . . . . . . . . . . . . . . . . . 213
Theorem 21.A (Sobolev embedding theorems). . . . . . . . . . . . . . . . . . . 238
Theorem 21.8 (The closed range theorem of Banach). . . . . . . . . . . . . 253
Theorem 21.C (The Riesz theorem on the characterization of infinite-
dimensional B-spaces) . . . . . . . . . . . . . . . . . . . . . . . . . . 255
Theorem 21.D (The Eberlein-Smuljan theorem on weak compact-
ness). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 255
Theorem 21.E (\Veak * compactness) . . . . . . . . . . . . . . . . . . . . . . . . . . 260
Theorem 21.F (Riesz-Schauder theory and Fredholm alternatives). 275
Theorem 21.G (Main theorem on the unique approximation-solva-
bility of linear operator equations). . . . . . . . . . . . . . . . 279
Theorem 21.1-1 (Refined Banach fixed-point theorem via interpola-
tion inequalities). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 286
Theorem 22.A (Quadratic minimum problems and the Ritz meth-
od) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 321
Theorem 22.8 (Method of orthogonal projection, duality, and a
posteriori estimates for the Ritz method). . . . . . . . . . . 326
Theorem 22.C (Linear strongly monotone operators and the Galerkin
met hod). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 340
Theorem 22.D (Fredholm alternatives for compact perturbations of
linear strongly monotone operators and the Galerkin
method). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 348
Theorem 22.E (Eigenvalue problems, the Courant maximum-mini-
mum principle, and the Ritz method) . . . . . . . . . . . . . 353
Theorem 22.F (Fredholm alternatives for Garding forms). . . . . . . . . 369
Theorem 22.G (Eigenvalue problems for Garding forms). . . . . . . . . . 369
Theorem 22.H (Regularity of generalized solutions for elliptic dif-
ferential equations in the interior of the domain). . . . 377
Theorem 22.1 (Regularity of generalized solutions for elliptic dif-
ferential equations up to the boundary) . . . . . . . . . . . 383
Theorem 23.A (Main theorem on linear first-order evolution equa-
tions and the Galerkin method) . . . . . . . . . . . . . . . . . . 424

1176
Theorem 24.A
Theorem 25.A
Theorem 25.8
Theorem 25.C
Theorem 25.D
Theorem 25. E
Theorem 25."
Theorem 25.G
Theorem 25.H
Theorem 25.1
Theorem 25.J
Theorem 25. K
Theorem 25. L
Theorem 26.A
Theorem 26. B
Theorem 27.A
Theorem 27.8
Theorem 28.A
Theorem 28. B
Theorem 29.A
Theorem 29.8
Theorem 29.C
Theorem 29.D
Theorem 29.E
Theorem 29.F
Theorem 29.G
Theorem 29.H
The()rem 29.1
Lisl of Theorems
(Main theorem on linear second-order evolution
equations and the Galerkin method). . . . . . . . . . . . . .
(Main theorem on the projection - iteration method
for k-contractive operators) . . . . . . . . . . . . . . . . . . . . .
(Main theorem on Lipschitz continuous, strongly
monotone operators and the projection-iteration
method). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Main theorem on minimum problems). . . . . . . . . . . .
(Main theorem on weakly coercive functionals). . . . .
(Main theorem on convex minimum problems) . . . . .
(Main theorem on monotone potential operators). . .
(Main theorem on pseudomonotone potential opera-
tors) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Main theorem on quadratic variational inequalities).
(The projection - iteration method for stationary con-
se r vat ion I a w s) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Main theorem on stationary conservation laws). . . .
(Main theorem of duality theory for stationary con-
servation laws and two-sided error estimates for the
Ritz method). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Convergence of the Kacanov method for variational
. I " )
Inequa Itles . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Main theorem on monotone operators). . . . . . . . . . .
(The generalized gradient method). . . . . . . . . . . . . . . .
(Main theorem on pseudomonotone operators). . . . .
(Main theorem on locally coercive operators) . . . . . .
(Abstract Hammerstein equations with angle-bounded
kernel opera tors) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Abstract Hammerstein equations with compact ker-
ne lope rat 0 rs) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
('redholm alternative for asymptotically linear, con1-
pact perturbations of the identity) . . . . . . . . . . . . . . . .
(Generalized antipodal theorem) . . . . . . . . . . . . . . . . .
(Fredholm alternative for asymptotically linear (S)-
opera tors) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Weak asymptotes and Fredholm alternatives) . . . . .
(Main theorem about nonlinear proper Fredholm
operators on B-spaces) . . . . . . . . . . . . . . . . . . . . . . . . .
(Nonlinear proper Fredholm operators on arbitrary
se t s) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Main theorem on locally strictly monotone Fredholm
operators) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
(Sufficient conditions for a strict local minimum via
locally regularly monotone operators). . . . . . . . . . . . .
(Sufficient conditions for a strict local minimum via
455
499
504
512
513
516
516
518
520
530
536
540
546
557
565
589
601
621
626
651
655
657
658
665
675
679
693

List of Theorems
Theorem 29.J
Theorem 29. K
Theorem 29.L
Theorem 29. M
Theorem 29.N
Theorem 30.A
Theorem 30. B
Theorem 30.C
Theorem 31.A
Theorem 31. B
Theorem J I.e
Theorem 32.A
Theorem 32.8
Theorem 32.C
Theorem 32.D
Theorem 32.E
Theorem 32.J.'
Theorem J2.G
ThelJrem 32.H
Theorem 32.1
Theorem 32.J
Theorem 32.K
Thel)rcm 32.L
Theorem 32. M
1177
linear eigenvalue problems-the abstract strong
Legendre condition and the abstract Jacobi equa-
tion). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 694
(Main theorem of abstract statical stability theory) .. 710
(Main theorem of bifurcation theory for Fredholm
operators of variational type). . . . . . . . . . . . . . . . . . .. 715
(Sufficient conditions for strict local minima in the
calculus of variations and stability) . . . . . . . . . . . . . .. 724
(Loss of stability and a general bifurcation theorem in
the calculus of variations) . . . . . . . . . . . . . . . . . . . . . .. 731
(A global multiplicity theorem). . . . . . . . . . . . . . . . . .. 735
(Main theorem on monotone nonlinear first-order
e v 0 I uti 0 n eq u a t ion s ) . . . . . . . . . . . . . . . . . . . . . . . . . .. ] 7 I
(Main theorem on semibounded nonlinear first-order
evolution equations) . . . . . . . . . . . . . . . . . . . . . . . . . .. 785
(Global solutions of the generalized Korteweg de
Vries eq ua t ion). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 792
(Maximal accretive operators and nonexpansive semi-
groups in H-spaces) . . . . . . . . . . . . . . . . . . . . . . . . . . .. 819
(Main theorem on quasi-linear evolution equations). 831
(Quasi-linear parabolic systems regarded as dynami-
cal systems). . . . . . . . . : . . . . . . . . . . . . . . . . . . . . . . . .. 834
{Main theorem on pseudomonotone perturbations of
maximal monotone mappings}. . . . . . . . . . . . . . . . . .. 867
(Maximal monotone mappings and IJammerstein
equations) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 873
(Maximal monotone mappings and elliptic varia-
tional inequalities) . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 875
(Maximal monotone mappings and first-order evolu-
tion equations) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 876
(Maximal monotone mappings and second-order evo-
lution equations) . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 879
(Regularization of maximal monotone mappings) . .. 881
(Characterization of the surjectivity of maximal mono-
tone mappings). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 886
(Main theorem on weakly coercive monotone opera-
tors) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 88 7
(The sum of maximal monotone mappings) . . . . . . .. 888
(Maximal monotone mappings and evolution varia-
tional i neq ual it ies) . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 894
(Convergence of the method of regularization). . . . .. 895
(Characterization of linear maximal monotone map-
pin gs ). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 897
(Extension of monotone mappings to maximal mono-
ton e map pin g s ). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 900

1178
Theorem 32.N
Theorem 32.0
List of Theorems
(The range of sum operators) . . . . . . . . . . . . . . . . . . .. 906
(3*-monotone mappings and Hammerstein equa-
tions) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 909
Theorem 32.P (Characterization of nonlinear nonexpansive semi-
groups on H-spaces) . . . . . . . . . . . . . . . . . . . . . . . . . .. 910
Theorem 33.A (Main theorem on nonlinear second-order evolution
equations) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 924
Theorem 33.8
Theorem 33.C
Theorem 33.D
Theorem 33.E
Theorem 33.F
Theorem 34.A
Theorem 34.8
Theorem 34.C
Theorem 35.A
Theorem 36.A
Theorem 36.8
(Quasi-linear symmetric hyperbolic systems of con-
serva tion laws) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 931
(Global existence and blow-up of solutions for or-
dinary differential equations in B-spaces) . . . . . . . . .. 941
(Blow-up of solutions for semilinear wave equations) 944
(Global solutions for the semilinear Klein-Gordon
eq u at ion). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 946
(Generalized viscosity solutions for the Hamilton-
Jacobi theory). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 951
(A-proper operators and the main theorem on sta-
ble discretization methods with inlJer approximation
schemes) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 966
(Main theorem on strongly stable operators) . . . . . .. 971
(N umerical range of nonlinear operators). . . . . . . . .. 975
(A-proper operators and the main theorem on stable
discretization methods with external approximation
sc hem e s ) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 983
(The antipodal theorem for A-proper operators) . . .. 1000
(A general existence principle for A-proper operator
equations). . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 1001

List of the Most Important Definitions
Monotone opera tor. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 54
maximal monotone. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 852
strictly monotone. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 54
strongly monotone . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 54
uniformly monotone. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 50 I
locally monotone. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 677
locall y regu la rl y monotone. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 692
A cc re t i ve 0 pe rat 0 r. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 820
maximal accretive (m-accretive) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 820
P se u do m 0 not 0 n e 0 pe rat 0 r. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 586
operator properties (M), (S), (S). t (P). . . . . . . . . . . . . . . . . . . . . .. 583,586
C oe r c i ve 0 pe rat 0 r . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 50 I
weak 1 y coerci ve . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 501
Proper operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 667
A - proper operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 965, 983
stable operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 50 I
strongly stable operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 50 I
Fredholm operator
linear. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 667
nonl i nea r . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 667
Index of an operator
linear operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
nonlinear operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
Compact 0J'Crator. . . . . . . . . . . . . :. . . . . . . . . . . . . . . . . . . . . . . . . · · . . .
Con tin uous operator. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
h . t .
emlcon Inuous. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
demicontinuous. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
667
668
597
597
597
597
1179

1180
list of the Most Important Definitions
weakly sequentially continuous. . . . . . . . . . . . , , . . . . . , . . . . , . . . . ,. 597
strongly continuous. . . . . . . . . . . . . . . . . , . , , , . , . . . . . , , . , . . . , , , ,. 597
Lipschitz continuous operator. , . , . , , . . . , . . . , . . , . . . . , , . . . , . . , , .. 597
k-contractive . . . . . . . . . . . . , , . . . . , . . . . . . . . . . . . . . . . , , . . , . . . , .. 597
nonexpanslve, . , . . . . . . . . . . . . . . . . . . . , , . . , . . . . . . . . . , . . , . . . . ., 597
Duality map
linear. . , . . . . . . . . . . . . . . , . . . . . . . . . . . . . . . . . . . . . . , , , , , . , . . . , .. 254
non Ii n ear . . . . . . . . . . . . . . . . . . . . , . , . . , . . . . . . . , . . . . . . . . . . . . , .. 860
Linear operator. . . . . . . . . . , . . . . . . , . . . , , . . , . . . . . . . . . . . . . . . . . . . . 9
positive (monotone). . , . . . . . . . . . , , . , . . . . . . . . . , . . . . , . . . . . . . , .. 264
strictly positive (strictly monotone) , . . . . . . . . . . , . . . . , , . . . , . . . . .. 264
strongly positive (strongly monotone). . . . . , . . . , . . . . , , . . . . . , . , .. 264
bounded. . . . . . . . . . . . . . . . . . , . . , . . . . . . . . , . . . . . . . . . . . . , . . . . .. 261
s ym met ric. . . . . . , , . , . . . . . . . . . . . , , . . . . . . . , . . . . . . , . . . . . . . . , .. 264
se I f - a d j 0 i n t . . , . . . , . . . . . , . . . . . . , . . . . . . . . . , . . , . . , , , . . . . . . . , . . 1 24
unitary . . , . . , . . , . . . . . . . . . . . . . , . . . . . . . . . . , . . . . . . . . . . . . . . . .. J 25
gra ph closed, , . . . . . . , . . , . . . . . . . . , . . . . . . . . . . . . . . . . . . . . . . . . . . 1 79
angle-bounded. . . . . . , , , . . , . , . . , . . . . , . . . . . , . . . . . . , . . . . , . . , .. 342
sectorial . . . . . . . . . . , , . , , . , . . . . , . . . . . . . . , . , . . . . . . , , . . . . . . . . . 170
Fractional power of a linear operator. . . . . . . . , . . . . . . . . . . . . . , 169, 1099
The J.-'riedrichs extension of a linear symmetric operator. . . . . , , . . . . . 126
Bilinear form . . . . . . . . . . . . , . , . . . . , . . . . , . . . , , . . . . . . . . . . . , , . , . .. 262
positive. . . . . . . . . . . . . . . . . . . . . . , . . . . . . . . , . . . . . . . . , . . . . . . . . .. 263
strictly positive. . . . . . . . . . . . . . , . . . . . . . . . . , . . . . , . . . . , . . . . . . . .. 263
strongly positive . . . . . . , . . . . . . . . . , . . , . . . . . . . . . . . . , . . . . . . . , .. 263
bo un d ed . , . . . . . . . . . . . , . . . . . . , . . . . . . . . . , . , . . . . . , . , . . , . . . . .. 263
compact . , . . . . . . . . . . . , . . , . . . . , . . . . . . . . , . . . . . . . . . . . . , . . . . .. 263
symmetric. . . . . . . . . . . . , . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 263
Garding form. . , . . . . . . , . . . . . . . , . . . . , . . . . . , . . . , . , , . . . . . . . . ., 364
Weak convergence. . . . . . . , . . . . . . . . . . . . , . . . , , . . . . . . , . . , . . . . . , .. 255
We a k. con ve r g en ce. . . . . . , . . , . . . . , . , . . . . . . , . , , . . . , . . . . . . . . . . .. 260
"unctional
Ii n ea r. . . . . . , . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . J 0
con vex. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 507
lower scmicontinuous . . , , . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 514
weakly sequentially lower semicontinuous. . , . . , . . . . . . . . . . . . . , .. 511
weakly coercive. . . . . . . . . . . . . . . . . . . . . . . . . . . , . . . . . . . . . . . . . . .. 513
Minimum of a functional
(s t ri c t) I oca I m i n i mum . . . . . , . . . . . . . . . . . , . . . . . . . . . . . . . . . . . . . .. 680
Evolution triple, . . . . . . . . . . . . . . . . . . . . . . . . . , . . . . . . . . . . . . . . . . . .. 416
Scmigroup . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . , 145
linear. . . . . . . . . . . . . . . . , . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 147
nonlinear . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 145
strongly continuous. . . . , . . . . . . . . . . . . . . . . . . . . . . . . . . . . . , . . . . . . 146
nonex pansl ve . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . , . . 146
analytic. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 147

List of the Most Important Definitions
1181
Generator of a linear or nonlinear semigroup . . . . . . . . . . . . . . . . . . . . . 145
Approximation methods
unique approximation-solvability. . . . . . . . . . . . . . . . . . . .. 279. 966. 983
consistency, stability, and convergence. . . . . . . . . . . . . . . .. 195. 966, 983
admissible i"'ler approximation scheme (Galerk in method). . . . . . .. 964
admissible e.ternal approximation scheme (difference method). . . .. 981
A-proper operator
with respect to an i,aner approximation scheme. . . . . . . . . . . . . . .. 965
with respect to an external approximation scheme. . . . . . . . . . . .. 983
Galerkin scheme. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 271
ba s is. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 27 1
complete orthonormal system . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 117
project ion opera tor. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 267
fi n i te elemen t . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 268. 330, 1036
ra pidi t Y of con vergence. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 280
Le be s g u erne as u re . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. I 0 1 0
Ualmost everywhere" . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 1011
Lebesgue integral of real and vector-valued functions . . . . . . . . . . . . .. 1014
Nem yck ii operator . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 561
The fundamental space Co(G) of test functions. . . . . . . . . . . . . . . . . . . . 18
D i s t rib uti 0 n s (ge n era I i zed fun c t ion s ). . . . . . . . . . . . . . . . . . . . . . . . . . . .. 1 04 7
Generalized derivative . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 232
So bolev space. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 236
In terpola t ion i neq ual i ties . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 283. 110 I
(Cf. also the List of the Most Important Definitions on page 862 of Part I.)

List of Schematic Overviews
Interrelationships between fundamental properties of linear and non-
linear operators. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 596
Two basic strategies: the idea of orthogonality and the idea of self-
d . · t 6
a OJn ness . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
Linear strongly monotone operators and their applications . . . . . . . .. 319
Basic strategies for nonlinear monotone operators. . . . . . . . . . . . . . . .. 470
Maximal monotone operators and their applications. . . . . . . . . .. 841. 842
Intcrrelationships between general principles of functional analysis and
the theory of monotone operators. . . . . . . . . . . . . . . . . . . . . 319, 842
Applications of monotone operators to nonlinear differential equations
and integral equations. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 902
The Pythagorean theorem in Hilbert space and quadratic minimum
problems (the Dirichlet principle) . . . . . . . . . . . . . . . . . . . . . . . . . 16
The Riesz theorem and its applications ..................... 6, 16, 319
Bounded and unbounded self-adjoint operators and their applications 104
The Friedrichs extension of linear symmetric operators and its applica-
tions to variational problems and linear and scmilincar partial
differential equations. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 104, 105
Necessary and sufficient conditions for variational problems ..... . . . 81
The final functional analytic formulation of fundamental classical the-
ories (Euler-Lagrange equation, Legendre condition, Jacobi's
eigenvalue criterion, Fredholm's alternative) . . . . . . . . . . . . . . .. 643
The methods of Ritz and Galerkin and their applications. . . . . . . . . . . 102
Stability of difference methods. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 207
Interrelationships between different notions of convergence in mea-
sure theory. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 1073
Interrelationships between different spaces in functional analysis (cr.
also the detailed schematic overview on page 752 of Part I). . .. 1077
1182

List of Important Principles
Four fundamental principles of modern analysis:
extension of operators via density. . . . . . . . . . . . . . . . . . . . . . . . . . . . . 70
co m pie t ion . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 95
smoothing. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 72
localization via partition of unity . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 79
Compactness principle: Each bounded sequence in a reDexive B-spacc
has a weakly convergent subsequence. . . . . . . . . . . . . . . . . . . . .. 255
Monotonicity
Four general existence principles:
the fixed-point principle of Banach and monotone opera-
tors. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 341, 475, 500
the fixed-point principle of Brouwer and Schauder and monotone
operators . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 470, 558, 589, 870
existence principle for variational problems via convexity and weak
compactness. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 512
Hahn- Banach theorem ......................... . . . . . . . . . . . . 176
the method of orthogonal projection (the Ricsz theorem) and linear
monotone operators . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 68
the justification of the Dirichlet principle. . . . . . . . . . . . . . . . . . . 60
the Friedrichs extension. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 126
Five general uniqueness prilJCiples and monotone operators. . . . . . . . . . 174
M olloto,aicit}' principle:
strict monotonicity implies uniqueness. . . . . . . . . . . . . . . . . . . . . . . .. 475
1183

1 184
List of Important Principles
monotonicity and coerciveness imply existence. . . . . . . . . . . . . . . . . . 558
the monotonicity trick for thc weak convergence of the Galerkin
method via maximal monotonicity . . . . . . . . . . . . . . . . . . . . . . .. 474
The fundamental principle of maxilnallllollololJicit}':
monotone hemicontinuous operators defined on the total B-space are
maximal monotone. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 474
convergence trick
for operator equations . . . . . . . . . . . . . . . . . . . . . . .. 474, 559, 585. 589
for c\'olution equations. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . , . . . . . 776
for the Y osida approximation (existence of nonlinear semi-
groups) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 478. 822
The fundamental importance of maximal monotone operators. . 841, 843ff
maximal monotone operators generalize both bounded and un-
bounded self-adjoint operators . . . . . . . . . . . . . . . . . . . . . . . . . .. 845
Coerciveness implies a priori estimates. . . . . . . . . . . . . . . . . . . . . . . . . .. 473
A priori estimates imply existence:
the Leray -Schauder principle via compactness. . . . . . . . . . . . . . . . . . 55
the Browder- Minty principle via lnol,olonieil}' . . . . . . . . . . . . . . . . .. 559
the Brezis principle via pseudomonotonicity . . . . . . . . . . . . . . . . . . . . . 589
strongly continuous perturbations of hemicontinuous monotone
operators are pseudomonotone . . . . . . . . . . . . . . . . . . . . . . . 582. 588
the method of cO"Jpen.4iated conJpaclness (cf. Chapters 62 and 86)
continuation of solutions of ordinary differential equations in
B-s paces . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 800, 941
Uniqueness implies existence
linear Fredholm alternatives . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 275t 348
nonlinear Fredholm alternatives. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 640
The Sard-Smale principle: proper Fredholm operator equations have
genericall}' only a /i'lite number of solutions. . . . . . . . . . .. 665, 672
Loss of stability can lead to bi/urcatioll. . . . . . . . . .. .. . . . .. . . . .. 641,686
J\'eeessar.\' conditions for minima:
the solutions of F(r4) = min! satisfy the Euler operator equation
f" ( II) = O. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 5 I 0
the c:onvexit}' of F is equivalent to the monotonicit}' of F' . . . . . . .. 509
Sufficient conditions for minima:
loea/I.v I,JOlJotone operators. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 690
locally regularly monotone operators (the generalized conditions of
Legendre and Jacobi) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 693. 694
General existence principles for minima. . . . . . . . . . . . . . . . . . . . . . 512) 513
Minimum problems and monotone operators. . . . . . . . . . . . . . . . . . . .. 516
Minimum problems and pseudomonotone operators. . . . . . . . . . . . . .. 518
Fixed-point principles:
for semilinear operator equations Lu = I{u). where the linear opera-
tor L is invertible (reduction to u = L -If(u)). . . . . . . . . . . . . . . .. 487
for scmilinear equations Lu = /(11). where L is IJOt invertible (equiva-
lent coincidence problems) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 644

Partial Differential Equations
1185
for semilinear evolution equations (reduction to a V oltcrra integral
equation via semigroups-mild solutions) . . . . . . . . . . . . .. 148,485
for quasi-linear evolution equations via propagators. . . . . . . . . . . . .. 149
Partial Differential Equations
Physical interpretation:
statio1Jary processes in nature lead to elliptic differential equations. . 22
dissipative time-dependent processes in nature (e.g., reaction. diffu-
sion processes) lead to parabolic differential equations. . . . III, 934
,,'are-propagation processes in nature lead to hyperbolic differential
equations . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 114. 934
Se,nigroups describe the causality of evolution processes in nature which
a re homogeneous in time . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. I 51
irrel'ersihle processes in nature lead to proper semigroups whose
generators are unbounded operators. . . . . . . . . . . . . . . . . . . . . . . 151
dissipative processes in nature correspond to nonexpansivc semi-
groups. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 154
The description of time-dependent processes by semigroups is more
natural than the use of classical partial differential equations.. 153
General information which can be obtained from partial differential equations
without solving them:
The importance of characteristics:
(i) Only noncharacteristic initial-value problems can be well-posed (cf.
Chapter 83).
(ii) Weak discontinuities of the solutions can only occur along characteristics
(cr. Chapter 83).
(iii) Strong discontinuities of the solutions are related to shocks (e.g., in gas
dynamics; cf. Chapter 86).
(iv) The discontinuities of the solutions are governed by the differential equa-
tions (e.g., the Rankine- H ugoniot jump conditions in gas dynamics;
cf. Chapters 83 and 86).
The importance of symmetries: Symmetries lead to conservation laws-
the Noether theorem (cr. Chapter 88)
Smoothness properties of the solutions:
the solutions of elliptic partial differential equations are smoother
the smoother the data are
linear elliptic cq uations. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 78. 376
nonlinear elliptic equations . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 88
the solutions of parabolic differential equations can be smoother than
the initial values. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 934
the solutions of hyperbolic differential equations arc never smoother
than the initial values. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 934

1186
list of Important Pnnclples
very smooth initial values for hyperbolic differential equations can
lead to strong discontinuities (e.g., formation of shocks in gas
d y na m i cs) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 935
Blow-up of solutions of evolution equations. . . . . . . . . . . . . . . . . . . . .. 937
The fu.ndamental importance of generalized solutions in mathematics
and ph y sics. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .
variational problems and partial differential equations . . . . . . . . . . . 3
semigroups and evolution equations. . . . . . . . . . . . . . . . . . . . . . . 153, 402
numerical methods (e.g., the Ritz and Galerkin method) . . . . . . .. 5, 330
The basic strategy of modern analysis:
existence of generalized solutions via functional analysis. .. 60, 103, 402
reglllar;t y of the generalized solutions. . . . . . . . . . . . . . . . . . .. 78, 88, 376
An important modern strategy- the use of several spaces:
refilled iteration method (boundedness of the iterative sequence (un) in
a "very nice" space and k-contractivity in a hpoorU space imply
. .,., ) 285
con vergence In a nice space ............................
rejlned Galerkin method (the solutions of the Galerkin equations
live in a "nice" space and they converge weakly in a "poor"
space) . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 602, 784
time-discretization (Rothe's method) combined with the refined
Galerkin method (cf. Chapter 83)
interpolation principles. . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 283, 1101
the Gagliardo- Nirenberg inequalities. . . . . . . . . . . . . . . . . . . . .. 286
the Moser-type calculus inequalities. . . . . . . . . . . . . . . . . . . . . .. 294
Approximation of Solutions
Fundamental approximation methods:
projection method (inner approximation scheme) . . . . . . . . . . .. 279, 963
Galerkin method (e.g., the Ritz method). . . . . . . . . . . . . . . . . .. 24, 102
difference method (exter'Jal approximation scheme) . . . . . . . . . . 193, 978
iteration method. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 495
project ion - i tera tion met hod . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 496
regularization method. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 477,895
Y osida approximation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 162, 822
maximal monotone operators . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 843
time-discretization (Rothe's method) (cf. Chapters 57 and 83)
The golden rule of numerical analysis: consiste1acy and stability imply
con t' e rye n C' e. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. I 9 5
difference met hod . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . 197, 978
linear operator equation. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 211
linear evolution eq uation . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 213
nonlinear A-proper operators with respect to an inner approximation
scheme (e.g., Galerkin method) . . . . . . . . . . . . . . . . . . . . . . . . . .. 966

ApprOXimation of Solutions
1187
nonlinear A-proper operators with respect to an e.'(ter1Jal approxima-
tion scheme (e.g., difference method) . . . . . . . . . . . . . . . . . . . . . .. 983
The rapidity of convergence of the Ritz and Galerkin method is
higher the smoother the solutions of the original equation
are. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 280. 323. 329
favorable smoothness properties of the solutions of elliptic and
parabolic equations. . . . . . . . . . . . . . . . . . . . . . . . . .. 78,88,376,934
unfavorable unsmoothness properties of the solutions of hyperbolic
differential equations. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 934
Duality and t'o-sided error estimates for the Ritl method. . . . . .. 335.537
Convergence of the Galerkin nJethod:
monotonicity trick for operator equations. . . . . . . . . . . . . . . . . . . . .. 474
monotonicity trick for evolution eq uations . . . . . . . . . . . . . . . . . . . .. 778
pseudomonotonicity trick for operator equations. . . . . . . . . . . . . . .. 589
uniqueness for the original equation implies convergence of the total
G a Ie r kin se que n ce. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 480
the condition (S)o implies the strong convergence of the Galerkin
sequence. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 585
refined Galerkin method with respect to several spaces
o pc rat 0 r eq u a t ion s . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 602
evolution equations. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 784
relined Galerkin method combined. with time-discretization (Chapter
83)
projection - iteration method . . . . . . . . . . . . . . . . . . . . . . . . . . . .. 499. 503
The method of regularization allows us to solve nonuniquely solvable
equations by means of a family of uniquely solvable equations. 894
Nonconstructive existence proofs can be converted into constructive
existence proofs by means of A -proper operators . . . . . . . . . . .. 96()
Consider the operator equation
Au = b,
U E X.
(E)
If the approximation method is consistent and stahle, then the following three
conditions are mutually equivalent:
(i) Equation (E) has a solution.
(ii) Equation (E) is uniquely approximation-solvahle.
(iii) The operator A is A -proper . . . . . . . . . . . . . . . . . . . . . . . . . . .. 966, 983

Index
The reader should also consult the detailed Contents of this volume and the index
material (List of Theorems, etc.). Moreover, the reader may also consult the Index of
Pan I. If several page numbers belong to the same catch word. then the primary
reference is italized. The page numbers 110 and 10 refer to page 10 of Pan I and the
present Part II. respectively.
Important properties of operators and sets arc summarized under the catch word
"operator'" and use"., respectively. See also the catch words M functional'" and Umulli-
t'alued mapping".
absolute continuit),
of integrals 1016
of mcasures l.Oll
accessory variational problem 
admissible triplet 184
a.c. (see almost everywhere)
almost e\'erywhcrc 1011
antiduality map 67
antipodal theorem 1700, 1000
generalized 
approximation methods (see method)
basic ideas of convergence m
approximation scheme
external 2&l
inner 964.970.972
approximation-solvable, uniquely (see
uniquely)
at almost all points (see almost
ever).w here)
atom UlZ6
axiomatics of limiting processes 12
Baire
category 1802
rlCld 1fi11
measure 1078
measu regular 1078
set 1fi11
Banach algebra 29tJ, 303
Banach fixed-point theorem 117, 319.
341
refined version 285
Banach space (B-space) 1768fT
basis of a space 271 If
beam 697.7S1. IV31Iff
bifurcation
and loss of stability 686
and the buckling of beams 697fT
basic ideas 1350ff, 683, 686, 698ff
for operators of variational type 712fT
for \'ariational problems 702. 730.0.
bifurcation point 1358
bifurcation theorems 712. 
1189

1190
bilinear form 262ff
bounded 263
compact 263
positive 263
strictly positive 253
strongl)' positive 263
symmetric 263
bloy,'-up of solutions 934. 937fL
bloy,'ing-up technique ill
Borel
field 1064. .uru
function 1078
measure 1078
measure. regular 1078
set 1.D11
boundary of stabilit)' 6&!
boundary value problem
first 21, 325ff
mixed 334, S22ff, 530. 538.0.
second 28. 331ff
third 28. 333ff
bounded nonlinearities lli
bounded variation Ull
branching equation 644. 
B-space (see Banach space)
buckling of beams 697ff
Caccioppoli set 1116
calculus of variations 21fT, SIn: 722ff
basic ideas 22. 33. 82
Campanato spare 91
Caratheodory condition 561. 571. 1.QlJ
Cauchy sequence 7
causality 150
characteristic ill
chemotaxis problem ill
closure of an operator 180
codimension 1765
coerci\'e functional ill
y,'cakly ill
coercive operator 501. 867,886
A-coercive 867
multivalued 867, 886
strongly 902
\\'eakly 501. 886
\\'ith respect to the point b 867
coerciveness condition
and a priori estimates ill
Inde
for partial differential equations ill
coin;dence degree 646. 736ff
collocation method 311
Colombeau algebra ill
compact (see operator or set)
compact embedding 237
compensated compactness (see also
Part V) 483. IV 26417
complete orthonormal system 117
completion principle 71, 95
complex interpolation method l.1.Q1
complexification 1770, 182
concave functional san
condition
(M), (S) (S) , (S)o, m
(P) S86
growth 
conservation laws (see a/so Part V)
521jJ. 535fT, 930fT, IV422
basic ideas 521ff
dual problem 537ff
Kacanov method 
projection-iteration meth(xI ill
Ritz method 537ft'
system of 2.ID
consistency 192, 195, 966, 2Rl
\\'eak 983. 222.
continuation method
for solutions of ordinary differential
equations in B-spaccs 8ooff,
941fT
for operator equations 112
for Euler- Lagrange equations 729.
IV224
in elasticity IV224
convergence
almost certainly 1072
almost cver)'where 1072
discrete 982
discrete. 982
in measure 1072
interrelations 1fi1J
of approximation methods m
stochastic 1072
strong con\'ergence (identical to
norm con\'ergencc) 1769, 7
uniform up to small sets 1070. 1.01J
vague 1.012
weak 255ft"

Index
\\'eak ·
weak-
784
260.447,1079
convex
functional 506
set 506
variational problem 81 ff, 5/6
convolution 1051
countable at infinity 1076
Courant
maximum-minimum principle 353ff
minimum-maximum principle 359
Crank - Nicolson method 194,205ff,
215fT
critical exponent 7541f, 1028
critically stable point 680
decomposition theorem 65
defect correction method 222
derivative
(-"'-derivative 1135
G-derivative 1135. 509
generaliled 61. 23/.ff. 299, 417fT
normal 20
of distributions 1048
of functions of bounded variation
1115
of vector-valued functions 176, 417D.
viscosity 950
difTeomorphism 1171
difTerence method I 92ff. 978ff. 988
difTerential inequalities 938fT
Dirac's delta distribution 1046fT
direct sum 1765, 266
orthogonal 266
topological 267
Dirichlet principle 11. 21, 400, 60ff,
399
Dirichlet problem 399
discretization method
general theory 959ff
stahle 965. 982
distribution (generalized function) 235,
418. /044fl
basic strategy 1045
regular 1047
tempered 1060
dual maximum problem 335, 386ff. 537
dual pair 598
1191
dual space 252
duality
in 8-spaces 251
in H -spaces 253
duality map 67, 254, 845.860
antiduality map 67
nonlinear 860ff
duality principle 386
effective domain 850
eigenvalue problem 32, 35U, 373
eigenvalues
comparison principle 355
upper and lower bounds 357,391
elliptic 525, 750
regularly strongly 366
strongly 366
weakly 525
elliptic differential equation
semilinear 487, 632, 661 fT. 745fT, 750,
754fT
quasi-linear 487,489.567, 590.610
strongly nonlinear 604
elliptic difTerential operator, proper 576
embedding (see also Sobolev embedding
theorems)
definition 237
important properties 265
energetic extension 126
energetic space 58, 60. 126. 128fT. 321
energy 22,167,457.933
entropy condition (see a/so Part V) 937
equicontinuity 189
equivalent
norm 8
scalar product 8
spaces 92
error estimates 280. 322. 344. 356, 361,
390,505.537,565
eigenvalue problems 355ff. 390fT
Euler equation 21, 86. 723
abstract 510
evolution equation
basic ideas 402jJ. 484fT
first order 423, 767fT. 876
quasi-linear 830
second order 453. 879, 923
semibounded 783fT

1192
evolution triple 404, 416
explicit difference method t 93, 204ff
extension of monotone operators 899
extension principle 70
for (unctions 305fT
F -derivative (Frcchet derivative) IUS.
F -space (Frechet space) liU6
finite clement method 27311: 330, 363.
1036ff
basic ideas 5, 273. 330
nO\\' ISO
formation of shocks ill
Fourier series 117ff
Fourier transform 1058
abstract 1090
and Sobolev spaces 290/f. 306ff,
IOS8ff
nonlinear &l1
fractal w.3
fractional powers of operators 168/f.
1099ff
Friehet-space (F-space) 1D.56
".redholm alternative
basic ideas 640fT
linear 120, 128, 275. 319. 347. 372
nonlinear 473. 489. 6400: 651. 6570:
663. 747. 1Sfi
Fredholm operator
linear 661
nonlinear 6fi1
typical properties 668ff,671fT
Friedrichs extension 6, 52, 126ff, 183
and interpolation theory 1109ff
and maximal monotone operators
821
basic ideas 103. 128ff
function (see operator)
functional
coercive ill
concave 507. 2.l6
convex 507,915
F -differentiable ll.11
G-differentiable 1135. 509
linear 10
lower semicontinuous 860
strictly convex SOl
\\'cakly coercive ill
Index
weakly sequentially lo\\'er
semicontinuous ill
functional calculus 138, I08Sff
and scmigroups 187
and unitary groups 186
basic ideas 106
simple variant 138
fundamental solution I052fT
Gagliardo-Nirenberg inequalities
2861: 1033
Galerkin equation (see Galerkin
method)
Galerkin inequality 862
Galerkin method 10lff
basic ideas of convergence ill
basic ideas of the method 101
convergence 279,339.347.402.424.
455. 499. 504, m 584. 589. 599.
621.655.771. 784,869,924. 963fT
convergence with respect to a "poor"
space 599, 1M
duality trick 344
refined error estimates 344
Galerkin scheme 2710: 1035ff
Girding form 364. 369ff
regular 364
GArding inequality 366, 393ff.lli
Gauss theorem 21
G-convergence 399
G-derivative 1135. jJll
Gelfand-Levitan-Maltenko integral
equation &W
generalized
boundary eigenvalue problem 3l
134,361
boundal)' value problem 21. 28.
132ff, 325D: 571ff
basic ideas 21 If
boundary values of functions 239,
ill22
initial-boundary value problem 427.
457. 780. 929
generalized derivative (see derivative)
and distributions 235. 418
generalized solution (see also generalized
problem)
basic ideas Iff, 21, 25ff. 63. 402ff

Index
evolution equations 142. 144, 148,
153, 169, 402ff, 767rr. 921ff
fundamental solution \'ia distributions
I052fT
history 2. 51fT. 86fT, 225ff
mild 107. 142, 148.153, 169, m
\'ia semigroups 153
viscosit)' 950
generator of a
semigroup J45, 154
nonexpansive nonlinear scmigroup
820. 911
gradient method 111252
generalized 564.ff, .568
graph 8.Si)
closed 179
norm 180
Green function 44. 53, 134
Green operator 323. 342
gro"'th conditions 491. 561. lli
importance of ID
relaxed 575
sublinear 754. 15&
supcrlincar 754fT
Hamilton-Jacobi equation
generalized solutions 94 7fT
Ilamiitonian system snz
Hammerstein equation 873, 908
basic ideas 61 SfT
Hammerstein integral equation g]JL
653
basic ideas 615ff
Hausdorff dimension Ull
Hausdorff measure 1111
normalized 1112
spherical lil2
heat equation III, 141 fT, 427, lDSJ
Hilbert space (H-spacc) 7ff
Hilbert space methods
basic ideas 3140", 402fT, 453fT
Hilbert's problcms 86fT
Hilbert-Schmidt theory 1/9ff.319
I-I-isomorphism 97
H-space (see Hilbert space)
Holdcr inequality 35, 410
with I-trick 37
'-folder space 1230, 1104
1193
homeomorphism 1755
homogenization 398
h)'perbolic differential equation
linear 456
quasi-linar 928fT
sem ili near 164
idea of orthogonalit)' 16. 68
identification principle 254, 4 t 2, 411
implicit difference method 194, 205fT
implicit function theorem I.1.SQ
variant of the ill
index 661
indicator funclion &58.
induced topology 1755
inequality
Bessel 117
Clarkson 257
differential 938fT
Ehrling 365
Gagliardo- Nirenberg 286jf. 1033
Gcirding 366, 368ff, 393. 425
Holder 35, 1020. 1072
Moser-type calculus 294ft"
Poincare 135
Poincare - Friedrichs 59
Sch\\'arz 8, 312
variational (see variational inequalit).)
Young 36,1J}2O
integral 101 J. 1071
equation 11 S. 349
operator 308
integration by parts 19, 1l1h
discrete 2S1
interpolation
couple 1102
inequality 283fT
Lagrange 268
interpolation theory 1101 fT
nonlinear 799. lli
irreversibility IS 1
iterated limits 190
iteration method 499. 
Jacobi equation 682ff. 686. 701. 725
abstract 
basic ideas 682. 6.8.6

1194
Jacobi's eigenvalue criterion 685. 694.
70 I, m
basic ideas 683. 685
Kaanov method S42ff
Klein-Gordon equation. semilinear
166,2M)
K-method in intcrpolation theol}'
1102
Korteweg-de Vries equation 792.
804ff
generalized 790fT
Laplace equation 43. 134ff, 331fT. 361fTt
1fiSJ
Laplacian 19
Lax pair 80S
Lcbcsgueintegral 1013 1071
Lebesgue measure 1010, 1068
Lebesguespace 236. 1019. 1071
discrete 286
of \'ector-\'alucd functions 406rr
Lebcsgue-Stieltjes integral ill&l
Legendre condition 81, 82ff, 694. 723ff
classical 82.8S
Legendre-Hadamard condition 85.
723ff
strong ill
lemma
Lax-Milgram 69
Morrey 91
variational 18. 77.41 S. 444
Weyl 78
Zorn 1511
life-span or solutions 938, 940. 945ff
linear subspace 1764. 9
local boundedness of monotone
operators SSS, 8M
local minimal point 680
strict 6&}
localization principle 79
locally coercive operators. main theorem
on 6!ll
locally uniformly convex B-space 256
majorant method 785/f. 
Index
majorizcd convergence 1015
mapping degree (see also Part I)
coincidence degree 646, 7 J6ff
ror A-proper mappings 998fT
for operators with condition (S)-t-
1003
maximal accretive operator 16O,81!l.
basic ideas 818ff
maximal monotone operator 843ff, 852
and skew-symmetric saddle functions
91 Sir
basic ideas 843ft'
pseudomonotone perturbation of a
866/f, ill
typical applications 846
typical examples 84Sff
typical properties 914tT
maximum principle 208rr
mean continuity lJ121.
measurable
function 1011, 1069
set 1010. 1069
measure 1064
absolutel)' continuous 1.Q15
Baire 1078
Borel 1078
complete ill66
continuous 1.016.
finite ill6S
Hausdorff 1111
nonatomic 1JU6
outer 1067
prcmcasure lfi6S
prod uct uru
pure point Im5
regular 1078
a.finitc 
singular Im5
space 1069
surface 1023. III S
vague convergence lD12
vector .11l16
\\'cak- convergence ffi12
zero 1!W}
(M)-condition m
method
collocation 311
continuation (see continuation
method)

Index
defect correction 222
difference 192/f, 978ff, 988
discretization 9S9ff
finite element (see finite element
method)
Galcrkin (see Galcrkin method)
gradient 565. 568. 111252
homogenization 398
Kaeanov 542ff
majorant 785ff. 9A3
orthogonal projection 335, 338, 388
overrelaxation 216
projection (see "projection method"
and MGaJerkin method")
projection-iteration 495fT, 499.. 504,
ill
reduction 27, 486ff, 689" 1.16
regulari7ation (see regularization
method)
Ritz (see Ritz method)
Rothe (see time-discretization)
secant modulus (see Kaeanov method)
Schmidt orthogonaJization J 91
time-discretization (see also Chapter
83) 484. 814
TrefTtz (see TrefTtz method)
truncation 870, 8.20
mild solution 107. 153" 165" 168fT, 
minimal sequence 58
minimal surface l1l1
. .
minimum
classical necessary conditions 82/f,
682ft"
classical sufficient conditions 682fT
local 6&}
necessary condition 517. 519.690
strict local 68n
sufficient condition 690" 693/f, 696.
701, 724, 726
minimum principle 511. 516
minimum problem
basic ideas 681 fT
convex 516.626
monotone operator
basic ideas 469fT
definition 54. j[J{!
history 4Off. 54, 86ff
main theorems 69, 339, 504. 516.
556,770. 867
1195
monotonicit)'
and contractivity ill
and convexity m
and uniqueness ill
condition 51.1
monotonicity trick ill
general 913
Moore-Smith sequence (see M-S
sequence)
Morrey space 92
Moser-type calculus inequalities 294.
125
M.S sequence (Moore Smith sequence)
1759
multigrid methods 221
multiplicity theorem 733ff, ill
multivalued mapping 8jOff, 912ff
A-cocrcive 867
bounded 913
coercive 886
cyclic monotone 8.S2.
demicontinuous 2.12
hemicontinuous 2.12
locally bounded 8M
maximal cyclic monotone 8S2
maximal monotone 852
monotone 852
pseudomonotone 913fT
strongly continuous 2.12
upper semicontinuous 2.12
natural boundary condition 29
negative norm 378, 1055
Nemyckii operator (see also Chapter 83)
298. 5610', 20j
noncoercive equations, basic ideas 640ff
norm 1768
normal derivative 20
normisomorphic 9 96
nullhomotopic US
numerical range of operators 2H
numerical stability
difference method 193ff. 207
Ritz method 392
p-measurable 1070
one-parameter group 146

1196
onc-parameter unitary group 147, 160
operator (see also "'functionar', "bilinear
form", and "multivalucd
mapping" jsee also Part I)
operator
A-coercive 867
A-proper 965.2&3
absolutc)' continuous 1Jl.12
accretive 160. B.2Jl
adjoint 124. 181. 253
analytic 1362
angle-bounded 342. 619ff. 
antilinear 10
asymptotically linear ill
B-compact 131
bilinear 262ff
bounded 9, 555 m
cocrci\'c (see coercive operator)
coerci\'e with respect to b 868
compact 153, 10, ill.
completely continuous 521
concave 
continuous 1770" 10, 596ff, 597. 1755
convex son
cyclic monotone 8..S2
demicontinuous 554. S21
dissipative 154, 159
dual 252ff
essential ID
essentiall)' self-adjoint 180
F-difTerentiable Illi.
Fredholm 1365, 665. 667ff
fundamcntal interrelations S26
fundamental properties 521
G-differentiable m
graph closed 179
hemicontinuous 554. 521
image compact (i-compact) S21
isometric 1089
k-contractivc .521
K-monotone m
linear 9. 261
Lipschitz continuous 521
locally bounded 555. ill
locally closed 612
locally Lipschitz continuous 521
locally monotone ill
locally regularly monotone 68.l
locally strictly monotone 611.
Index
locally strongly monotone 611.
lower semicontinuous 1456, 86a
maximal accretive 160. &2fi
maximal cyclic monotone 8.S2
maximal dissipativc 160
maximal monotone 820, 852
measurable lOll, 1069
monotone 54. 500. 5.26
n-monotone 901
Nem)'ckii (see Nemyckii operator)
nonexpansive 521
of bounded variation 
of variational type 11.3
piecewise continuous 1082
p-measurable 1070
positive 263
potential son
proper S21
pseudomonotone 586.. 867
sectorial 170, 1096ff, 1099
self-adjoint 124
semibounded ill
semimonotone 6.lil
skew-adjoint 124
skew-symmetric 119
stable 481 9 jJJ1
strictly convex S01
strictly monotone 54, SOU
stricti)" positive 264
strictly sectorial 1097
strongly coercive 902
strongly continuous 555. m
strongly monotone 54. sm
strongly positive 264
strongly stable Sill.
symmetric 119. 179. 264
3-monotone 901
3*-monotone 901
3-a-monotonc 901
unbounded 9
uniformly continuous S21.
uniformly monotone 481 501
unitary 97. 125. 1089
weakly coercive 501. 513.886
weakly continuous 1755, 1779ff, 
weakly sequentially continuous 261,
296, 297,j2Z
\\'cakly sequentially lower
semicontinuous ill

Index
order cone 1276
orthogonal complement 65
orthogonal projection 65, 269ff
orthonormal system t 16
overrelaxation method 216
parabolic differential equation
linear 141,426, 1098
quasi-linear 119. 829, 832 9 811
semi linear 171
parallelogram identity 16" 56
parameter integral 1018
Parsc\'al equation t 18
partial regularit)' 90, llll
partition of unit)' 19
periodic solutions 739 9 811
perpendicular principle 16. 65
piecewise continuous function 1082
piecewise smooth boundar)' 18
Poincarc- Friedrichs inequality 59
point
liticaJly stable 6&l
regular 668
singular (critical) 668.
stable 68D
stationary 1Q6
unstable 6.80
weakl)' stable 6&l
Poisson equation 43. 132fT, 325fT. 361ff
Polish space 1762, ill11
potential operator S!l6
generalilJ:d ill
monotone lli
pseudomonotone 515" ill
Pre-Hilbert space 7
prcmcasurc 1D6.S.
principle of stationary action (see also
Part V) 708. 948. IV69ff
product measure lO1J
projection method (see also Galerkin
method) 112, 115, 279
projection operators 276fT
con\'ergence of 280. 976
orthogonal 267fT
projection-iteration method 499. 
basic ideas 496ft'
for conservation laws 521
propagator 149fT
1197
proper subregion 72
propcrncsstrick 
pscudomonotone operator SM
basic ideas 581 IT
pscudorcsolvent 
quadratic minimum problem 56, 320.0:
389
duality principle 386
quantum physics (see also Part V) 811.
1092. IV112ff
and spectral thoor)' 1093
radially s)'mmetric solution m
range 8SO
of sum operators 906. 917
rank 661
reaction-diffusion process m
reactor technology 218
reduction principle 27, 4866, 689. 116
reflexive 252
region 1758. 19
regu lar variational problem 81. 83
regularity of generalized solutions 5.
64. 78. 327. J16.ff
regularity theory 88/f. 327. 3766
basic strategies 90, 327
regularization (see aho Yosida
approximation)
basic ideas 477" 8.SJl
elliptic 
method 868. 
of maximal monotone operators W
of pseudomonotone operators 88J
parabolic 
regularly elliptic 81" 83, 85
resolvent 1795. 1091
resonance 663
at the first eigenvalue H6
restriction operator 964, 980, 22(l
Ricsz theorem 6. 16.66. 130fT, 255. 319
Riesz..Schauder theory 275
Ritz equation (see Ritz method)
Ritz method (see also Galcrkin method)
basic ideas 4. 24jf. 31 fT. 320. 329
conservation laws 531ff
convergence 320,329.356

1198
Ritz method (continued)
convex problems 111250
duality and a posteriori estimates
335. ill
duality and the Trcmz method 336,
386, 539. 111502
duality trick and refined error
estimates 335. ill
eigenvalue problems 31ft. 356JJ: 361ft
examples 93, 329ff, 363, 392, 537ff
finite clements 331. 363, 1036ff
general duality theory 111499.
1I1502ff
numerical stability and strongly
minimal systems 392
two-sided error estimates (a posteriori
error estimates) 335. 357, 391,
j3tT
Rothe's method (time-discretization,!
see also Chapter 83) 484. 814
saddle function, skew-symmetric 21.6
saddle point 111292. 111458
scalar (inner) product 7
scattering of particles (see also Part V)
8.1fi
Schmidt ortbogonalization method
191
Schrodinger equation &11
Sch\\'arz inequalit), 8
generalized 312
(S)-condition m
second variation 68R
regular 622
self-adjoint operator
basic properties 124, 181ff, 828, 1.08J
functional calculus 138,I085jJ
simple variant of functional calculus
138
scmiboundcd evolution equations. main
theorem on ill
seminow 150. 8.Y
scmigroup 146ff, 817ff. 1093ff
analytic 147. 1096. 1099
basic ideas 145ff, 818ft
bounded analytic 147, 1099
linear 147, 1093
Index
nonexpansivc 146, 1591f, 820. 838.
910, 1094ff
parabolic equations 153jf, 829. 1098
strongly continuous 146
uniformly continuous 146, 1094
semilinear equation
basic ideas 148.0: 
elliptic 487. 661. 750. 7540'
hyperbolic 164jf. 
parabolic 168ff
&:hrodinger 167
seminorm 1768
separable 9
set (see also Part I)
absorbing .l.QS1
algebra 1064
bounded 9
Caccioppoli 1.1.1.6
circled lOS1
closed 1751. 9
compact 1756. 9
connected 1757
convex S01
cylindrical 1074
dense 1751, 9, 70
measurable 1010. 1069
monotone ill
nowhere dense 175 I
of first Bairc category (meagre) 1802
of second Baire category 1802
open 1751, 9
relatively closed 1755
relatively compact 1756, 9
relatively open 1755
a-additi\'ity 1064
a-algebra 1064
7.ero lil65
single step method 216
smoothing operator 730'
smoothing principle 72
Soboley constant
best m
SoboJe\' embedding theorems 2370:
10260'
basic ideas 239
for evolution equations 450
general variants 1026fT
simple variants 185, 244

Index
Sobole\' space 4, 61, 235ff, I025ff
abstract 169. 1100
and Banach algebras 292ff,.lJlY
and distributions 1055
and Fourier transform 290jf. 306fT.
1058fT
basic definitions 235
basic ideas 61. 239ff
critical exponent 754./f. 1028«
density theorem 238, 304, 1fi2S
difference approximation 374
discrete 98Sff
embedding (see Sobole\' embedding
theorems)
equivalent norm 238.0: 306. lOJ2
extension operator 306, 1fi2S
finite elements 273, 1036fT
fractional order 1O.JL. 1061
Gagliardo- Nirenberg inequalities
286ff
general 
Galerkin schemes 1035«
history 51, 226
interpolation inequalities 284. 
interpolation operator 1.1lY
interpolation theor)' II04ff
Moser-type calculus inequalities
294fT
of vector-valued functions 422, 446
regularity theory 377ff
summary of properties 1025ff
weakl)' sequentially continuous
operators 296
soliton 802
space (see also Part I)
Banach (B-spaoe) 1168ff
Besov lJ1}5
Campanato 91
Frechet wn
Hilbert (H-space) 7
Holder 1230
Lebesgue 236, 1019ff
locally compact ill16
locally convex 1779. ill.S6
Morrey 92
of functions of bounded variation
l.lH
normal 1596, 11111
1199
Polish 1762.1fi21
probability 1074
Sobole\' (see Sobolev space)
Sobolev-Slobodeckii 1061
topological 1750
topological vector 1768
Triebcl- Lizorkin lJ.fi.S
spectral
family W&I
radius 1795
theory 1794. 1083«
spectral transform 803ff, &12
inverse 804. 801
physical interpretation 8.10
spectrum 1795, 1091
absolutely continuous ill22
classification 1091
continuous 1091
discrete 1091
essential ill22
physical interpretation 1m2
point 1091
pure point 1091
purel)' continuous 1091
singular ill22
stability
basic ideas 682. 685ff
elasticit)' 370
elliptic equations 370
numerical 193, 195, 966, 28.1
stability of difference methods 192ff.
195jf
von Neumann's test 219
stability theory (see also Parts I, IV. V)
680. 682ft'. 686. 697«. 701 fTt 709.
7300
abstract statical 709. ill
stable homotopy 747ft'
stable operator 5.O.l
basic ideas 
stable point 680
star-shaped ill.
stationary point 206
step function lOll
Stieltjes
integral l!l8.1
measure 1068
operator integral 10M

1200
stitt differential systems 221
stochastic convergence I072fT
strategies 469ff
for c\'olution equations 402.0: 484ff
for solving nonlinear partial
differential equations 483ff
for the theory of monotone operators
469ff
st riet
inductive limit 1fiS1
local minimal point 680
local minimum 6&}
minimum 320, 6Bf1
strictly convex
B-space 256
functional sn6.
string. nonlincar ill
strongJ)'
elliptic s)'stem 396
minimal Ritz system 393
stable solution 12A
strongl)' elliptic differential equation
366ff, 371 ff, 396fT
regularly 366
subgradient 856ff,111385
sublincar growth 754, 158
submanifold IVSS6
sum trick 38
supcrlinear growth 754ff
support 1048
surface integral W21
surface measure W21
generalized 1.1.1.5
surjectivity of maximal monotone
operators 886
suspension 
symmetric h)'perbolic system
nonlinear (see Chapter 83)
quasi-lincar 2.3.Q
synchronization condition 980. 9!ll
tensor product 1050
theorem (see also lemma)
Amann 621. 626, 8.Y
-
Ambrosetti and "rodi ill
antipodal 1700, 655. 968, 1000, lOO!!
Bairc-HausdorfT 1776, 1802, W
Banach 253.470
Index
Banach-Steinhaus 1777. 258. 261.
608, 201
Bolzano ill
Bourbaki Kneser 1504
Brczis S89 897
Brczis-Haraux 206
Brouwer 151. 470. W
Browder 867, 886, 21!
Browder-Gupta 62.l
Browder-Minty ill
Calderon-Zygmund 6J2.
Carathcodory 
closed range 1777. 253
Crandall-Lions 951
Crandall- Pazy 2.J1l
Debrunner-Flor 162, W
DeGiorgi- Nash 89
Dini 190
Eberlein-Smuljan 255
Egorov 1013. 1070
Ehrenpreis- Malgrange 1052
Friedrichs 126
Fubini 1017, 1014
Hahn-Banach 1776,176, IIIJ70ff
Hausdorff m
Hess 626. ill
.Iess-Kato 6D1.
Hcstcncs 365, 389
Hilbert l!l8!
Hille- Yosida 160, 1094
implicit function 1/50 m
invariancc of domain 1705, 968,
1001
Jackson 272
Kacurovskii ill
Kadec- Troyanski 862
Kantorovic 211
Kato 613. 8..ll
Kato-Lai 1Sj
Klaincrman 
Komura &.l2
Landesman-Lazer 663. 150
Lax 211 t 213
Lebesgue 443. 1011.1015,1018,
1.Q1S
Leray- Lions ill
Ljapunov lO16
Luzin Ull.l
Majda ill

Index
Minty 855
Moser 6ll
Neeas 6S8
open mapping 1777
nonlinear l.OO6
Pctrysh)'n 966
Pettis 1012
PohoZaC\' 156
Prohorov 1080
Rabinowitz ill
Radon-Nikodym .1fi1S
Rellich 135
Riesz (Fryges) 6. 16. 66. 255, 319
Riesz (Marcel) 1103
Riesz- Kolmogorov l.Q2J.
Riesz- tvlarkov 1!l12.
Riesz-Schauder 275
Rockafellar 860. 881. 888
Sard-Smalc 612
Schauder 156.253.945
Stone 116, 161
Tocplitz S4&
Tonclli l1U 7. 1074
uniform boundedness 1777.2Q1
Visik ill
Vitali l!tl1
Vitali Hahn-Saks 1ill1
von Neumann 1084, 1089
Weierstrass ill
Zarantonello 504. 915. 975
theta function 8n2.
time-discretization (Rothe's method) (see
also Part V) 484. 814
topological vector space 1768
topology ill
induced 1755
"'eak 1779
total step mcthod 216
TretTtl method 336. 386. 539. 111502
triangle inequality 8
trick of
contractivity 475. 
con\'ergence of approximation
mcthods 479fT
equi\'alent fixed-point problem 485fT
maximal accretivity m
maximal monotonicity m
monotonicity 474. 913
properness m
1201
red uct ion 27, 486, 689. 716
stable operators 
Toeplitz 
Yosida approximation 102, 478. 822.
1094
truncation method 870. 820
t"'o-sided error estimates 326, 336,
357, 390. 391. ill
uniform boundedness principle (see
theorem of Banach-Steinhaus)
uniform con\'ergence 189
uniforml)' con\'cx B-space 256
uniquely approximation-solvable
linear 279
nonlinear 960,. 965, 2S.l
uniqueness principles 174
unstable point 6BD
"ague topology 1080
value
regular 668
singular (critical) 668. 
variation 23, 6BB.
regular 622.
variational inequality (see also Parts I.
III, IV, V) 507. 510. 519. 527.
------
au
evolution S2J
Kanov method j!S
variationallcmma 18. 77, 415, 444
variational problcm 21 fT, 81jJ. 722ff
basic ideas 21 If, 81 fI'
viscosity
artificial 
derivative 950
solution 950
wave equation 113, 14Jff, 456, 944,
1JW
semilinear 932, 
with nonlincar friction 228
weak asymptotes 658
weak convergence 255fT, 258
and wcak topology 1779
y,'eak. convcrgence 260fT

1202
weak I)' sequentially lower semi-
continuous ill
weak)' stable point 68D.
well-posed problem 195.212
\'amabc problem (see also Part V) 755,
152
Yang-Mills equation (see also Part V)
ill
Index
Y osida approximation
basic ideas 418.
linear 162. 1094
multivalucd operators 111570
nonlinear 822
Young inequality 36.1n2D
zero
measure Ull1l
set illM

This volume is devoted to the theory of nonlinear monotone opera-
tors. Among the topics are monotone and maximal monotone
operators pseudomonotone operators potential operators,
accretive and maximal accretive operators, nonlinear Fredholm
operators, and A-proper operators along with extremal problems,
nonlinear operator equations, nonlinear evolution equations of first
and second order, nonlinear semigroups nonlinear Fredholm
alternatives, and bifurcation. The book also emphasizes the
methods of nonlinear numerical functional analysis The applica-
tions concern variational problems nonlinear integraJ equations
and nonlinear partial differential equations of elliptic, parabolic,
and hyperbolic type, including approximation methods to their
solution For the convenience of the reader, a detailed Appendix
summarizes important auxiliary tools (e.g., measure theory, the
Lebesgue integral. distributions, properties of Sobolev spaces
interpolation theory, etc.). Many exercises and a comprehensive
bibliography complement the text.
The theory of monotone operators is closely related to Hilbert's
rigorous justification of the Dirichlet principle, and to the 19th and
20th problems of Hilbert which he formulated in his famous Paris
lecture in 1900, and which strongly Influenced the development of
analysis in the twentieth century.
ISBN 0-387-9 167-X
ISBN 3-5 0-9716 -X