A series of Journals publishing the most significant developments in the important research areas ol Soviet
science. In making these publications available, the publisher hopes to contribute to the further development
of international cooperation between scholars and to a greater understanding among scientists.
ADVISORY EDITORS
P. Carruthers M. Longair
Los Alamos National Laboratory Royal Observatory
New Mexico Edinburgh
p. Doty S. Ichtiaque Rasool
Harvard University NASA
Massachusetts Washington D.C.
V.I. GoI'dansWr RX Sagdeev
Institute of Chemical Physics Institute for Space Research
Moscow Moscow
M. Levy
Untversite Pierre et Marie Curie
Рапа
MATHEMATICAL PHYSICS REVIEWS
Edited by S.P. Novikov, Landau Institute of Theoretical Physics, Russian Academy of Soiencee, Moscow.
Section С of Soviet Scientific Reviews
GENERAL INFORMATION
Alma and Scope. PHYSICOCHEMICAL BIOLOGY REVIEWS publishes review articles covering significant
developments In Soviet research, written by eminent experts In the field.
К is intended io make accounts of recent scientific advances in Russia readily available to Western scientists
who do not read Russian.
© 1992 Harwood Academic Publishers GmbH. All rights reserved.
No part of this publication may be reproduced or utilized in any form or by eny means, electronic or mechanical,
including photocopying and recording, or by any information storage or retrieval system, without permission
In writing from the publisher.
ORDERING INFORMATION
Each volume is comprised of an Irregular number of parts depending upon size. Issues are available Individually
as well as by subscription. 1S92 Volume: 9
Orders may be placed with your usual supplier or directly with Harwood Academic Publishers GmbH, c/o STBS
Ltd (distributor for Harwood Academic Publishers GmbH), P.O. Box 90, Reading, Berkshire RG1 8JL, UK or
P.O. Box 786, Cooper Station, New York, NY 10276, USA. Journal Subscriptions are scld on a volume basis
only. Clalnw-tor nonreceipt of Issues should be made within three months of publication of the issue or they
will not be honored free of charge. Subscriptions are available for microform editions. Details will be furnished
upon request
SUBSCRIPTION RATES: Base list subscription price per volume: ECU123 (US$118; Dfl289). * Available only
to single users whose library already subscribes to the journal OR who warrant that the journal is for their
own ues and provide a home address for mailing. Orders must be sent directly to STBS and payment must
be made by personal check or credit card. 1
Separate rates exist for different users such as academic and corporate institutions. These rates may also
Include photocopy license and postage and handling charges. Special discounts are also available to continuing
subscribers through our Subscriber Incentive Plan (SIP).
•The European Currency Unit (ECU) is the worldwide base list currency rate; payment can be made by draft
drawn on ECU currency in the amount shown. Alternatively, the US Dollar rate applies only to North American
subscribers; the Dutch Guilder rate applies only to UK and Mainland European subscribers. Subscribers from
other territories should contact their agent or one of the STBS Subscription Departments:
(continued on Ihe inside back cover)

PERTURBATION THEORY IN
PERIODIC PROBLEMS FOR
TWO-DIMENSIONAL
INTEGRABLE SYSTEMS
Contents
Introduction 3
1. Perturbation Theory of Finite-Zone Solutions of Evolution
Lax-Type Equations 8
2. Spectral Theory of Non-Stationary Schrodinger Operators 38
3. Periodic Problem for Kadomtsev-Petviashvili-Type
Equations 73
4. Spectral Theory of Two-Dimensional Periodic Schrudinger
Operators 85
References 99
Index 103
GEOMETRIC INTEGRATION THEORY
ON SUPERMANIFOLDS
Corrigendum 105

Sov. Sci. Rev. C. Math. Phys. Vol. 9, 1992, pp. 1-103
Photocopying permitted by license only
© 1992 Harwood Academic Publishers GmbH
Printed in the United Kingdom
PERTURBATION THEORY IN PERIODIC
PROBLEMS FOR TWO-DIMENSIONAL
INTEGRABLE SYSTEMS
I.M. KRICHEVER
L.D. Landau Institute of Theoretical Physics, USSR Academy of Sciences, Moscow
ABSTRACT
The perturbation theory of finite-zone solutions of two-dimensional
integrable equations is developed. As a special case the theory of
perturbations of finite-zone solutions of one-dimensional evolution
equations of the Lax type (the Korteweg-de Vries and Sine-Gordon
equations) is considered. The spectral theory of two-dimensional
periodic operators is surveyed.

INTRODUCTION
Despite its diversity, the range of problems to which the present article
is devoted can be unified within the framework of perturbation theory.
In fact only some of the problems in question are directly related to the
construction of the theory of perturbations of periodic solutions of
two-dimensional integrable systems. For other problems, the ideas and
methods of perturbation theory are used as the basic tool to solve the
problems.
Each perturbation theory begins with the answers to the questions
'What to perturb?' and 'How to perturb?' In our case the answer to the
former question is provided by the construction of periodic and quasi-
periodic solutions of spatially two-dimensional equations admitting a
commutator representation of the form
ИУ - L, 3, - A] = 0, A)
where
L = 2 щ(х,у, t)d\, A = 2 Ф'У< Wl B)
are differential operators with matrix or scalar coefficients. The con-
construction ([1, 2]) is based upon the notion of the Baker-Akhiezer-
Clebsch-Gordan function Ф(х,у, t, Q), which is equipped with specific
analytic properties for QeP, Г being an auxiliary algebraic curve.
These analytic properties constitute a natural generalization of the
analytic properties of the Bloch functions of one-dimensional periodic
finite-zone operators, which were established in a series of articles by
Novikov, Dubrovin, Matveev, and Its (see [4, 5] for a survey of these
articles).
From the point of view of the problem of constructing solutions of
non-linear equations, it was sufficient to solve the algebraic-geometric
inverse problem for finite-zone operators
(аду-1)ф(х,у, t, Q) = 0, (д<-А)ф(х,у, t,Q) = 0 C)
even without posing the direct spectral problem. In addition, such an
approach left open the question of the role and position of the
solutions constructed for the periodic problem for spatially two-
dimensional equations of the Kadomtsev-Petviashvili (KP) type.

4 I.M. KRICHEVER
For equations of the Lax type in the one-dimensionai case
Lt + [L,A]=0, D)
the existence of both the direct and the inverse spectral transformations
for the operator L with periodic coefficients enables one, in principle,
to prove that the set of finite-zone solutions is dense among all smooth
periodic solutions (however, this has not always been proved up to the
level of rigorous mathematical theorems). In the two-dimensional case
the situation turns out to be much more complex.
For example, for the KP equation
0,о2 = ±1, E)
which admits of representation A) (which was found in [6, 7]) with
L = d2- u(x, y, t),A=- d3x + { ud% + w(x, y, t) F)
the answer differs substantially for the two versions of the equation,
namely the KP-1 equation (a1 = - 1) and the KP-2 equation (a2 = 1).
As shown in [8], the periodic problem for the KP-1 equation is not
even formally integrable. It was shown in [9] that the same problem for
the KP-2 equation is integrabie and each smooth periodic solution of
the equation can be approximated by finite-zone solutions (locally, this
was proved by the present author in [10, 11]).
The assertion follows from the spectral theory of the operator
M = adr- d1 + u{x, y), Re а Ф 0 G)
with a periodic potential u(x,y), the construction of which is given in
Section 2.
It is impossible to find the answer to the question 'How to perturb?'
without constructing the spectral theory of operators of the form
аду - L. It is obvious that to construct the perturbation series with the
main term being some known exact solution, it is necessary to know the
'neighbourhood' of the solution in the space of all periodic solutions,
i.e. it is necessary to know a complete basis of solutions of the
linearized equations. For example, the spectral theory of the operators
G) with finite-zone potentials ио(дг, у, t) enables one to pro^e that for
ail / of the solutions of the linearized KP-2 equation
O = 0 (8)
presented in Section 3.1 form a basis in the space of square-integrable

PERTURBATION THEORY 5
periodic (with respect to x and y) functions. If the basis in known, one
can easily write down an asymptotic solution of the form
u(x,y, t) = uQ(x,y, t) + 2 «Ч(-г,.У, t) (9)
i-i
both for the KP-2 equation itself and for its perturbations (e is a small
parameter). Just as in the case of the multiphase non-linear WKB
method (the Whitham method; see [12, 13]) in the spatially one-
dimensional case, the requirement that already the first term of (9) be
uniformly bounded implies that the parameters /,, . . ., /N for a finite-
zone solution should depend on the 'slow' variables X=ex, Y= ey, and
7*=e/. The equations describing the slow modulation /k = Ik(X, Y, T)
are called the Whitham equations. For spatially two-dimensional
systems the equations were obtained for the first time in [14], the
results of which are presented in the final parts of Section 3. For these
equations, which constitute a system of non-linear partial differential
equations on the TeichmQiler space, a construction of exact solutions is
presented. In one spatial dimension the construction gives an effective
formulation of the scheme of [15], where a generalization of the 'hodo-
graph' method for solving 'diagonizable' Hamiltonian systems of the
hydrodynamic type was proposed. (The theory of Hamiitonian systems
of the hydrodynamic type was constructed in A6, 17].)
As an important special case in which the results can be applied, the
construction of the solution of the Khokhlov-Zaboiotskaya equation
1<Л|„ + ЭжA1|-|и11ж)-0, A0)
which is well known in the theory of non-linear wave beams, is
presented separately in the final part of Section 2. (A detailed biblio-
bibliography of works devoted to this equation can be found in [18].) Note
that A0) is the quasiclassicai limit of the KP equation.
As already mentioned, the spectral theory of operators of the form
G) constitutes the foundation of the theory of perturbations of
periodic finite-zone solutions of the KP-2 equation. At the same time,
the approach proposed for constructing the theory is based on the ideas
and methods of the theory of perturbations.
The construction of an effective spectral theory of the Sturm-
Liouville operators undertaken in the above-mentioned articles by
Novikov, Dubrovin, Matveev, and Its led to a new insight into the

6 I.M. KRICHEVER
whole approach to the construction of the spectral theory of arbitrary
one-dimensional linear operators with periodic coefficients.
The assertion that the Bloch functions of such operators for
arbitrary complex values of the spectral parameter E are the values
assumed on different sheets of a Riemann surface by a single-valued
function (on that surface), which appears now to be an obvious fact,
remained within the framework of Floquet's classical spectral theory.
It turned out that the^analytic properties of the Bloch functions on the
Riemann surface are fundamental for the solution of the inverse
problem of reconstructing the coefficients of operators from spectral
data. In the case where the Riemann surface is of finite genus, the
solution of the inverse problem is based on the apparatus of classical
algebraic geometry and the theory of theta functions. (The generaliza-
generalization of the algebraic geometric language and theta functions to the case
of a hyperelliptic curve of infinite genus corresponding to the Sturm-
Liouville operator with a general periodic potential was obtained in
[19].)
In an unpublished paper by I.A. Taimanov it was proved using
methods which are completely analogous to those of [20] that the Bloch
functions for the operator M with a smooth real-valued periodic
potential, defined as those eigenfunctions of the operators of transla-
translation by the periods with respect to x and у that satisfy the equation
Мф - 0, can be parametrized by the points of the Riemann surface Г
(as in the one-dimensional case). Moreover, the multipliers wt(Q) and
wi(Q)> which are the eigenvalues of the monodromy operators, turn
out to be holomorphic on the surface. The proof is based on the
Keldysh theorem on the resolvents of a family of absolutely continuous
operators depending holomorphically on some parameters. Unfor-
Unfortunately, within the framework of this approach one fails to obtain any
detailed information about the structure of Г, which is necessary to
prove the theorem on approximation.
The approach to the construction of the Riemann surface for the
Bloch functions proposed in [9] has a constructive character and is
more effective. In part 2 of Section 2 we construct formal Bloch
solutions with the aid of certain series which are similar to the series
used in perturbation theory. In the following part of Section 2 it is
proved that the series converge in various domains. The domains can
next be 'pasted together' to yield a global Riemann surface. It turns out
that outside any neighbourhood of 'infinity' the surface is of finite

PERTURBATION THEORY 7
genus. Roughly speaking, this is the condition that makes it possible to
approximate an arbitrary potential by finite-zone ones, i.e. by
potentials such that the corresponding Riemann surfaces are of finite
genus.
The final Section of the paper is concerned with the spectral theory
of the two-dimensional periodic Schrddinger operator
tf0 = Э,2 + д;г + u{x,y). A1)
The inverse problem for the two-dimensional Schr6dinger operator
with magnetic field
Я = (dt-iA&.y)I + (ду-1А2(х,у))г + u(x,y) A2)
based on the spectral data corresponding to a single energy level E=E0
was posed and discussed in [21]. In this article the class of 'finite-zone
operators at a given energy level' was constructed. From the point of
view of spectral theory, the class is determined by the fact that the
Riemann surface for the Bloch functions corresponding to the given
energy level, known as the 'complex Fermi curve', is of finite genus.
In [22, 23] conditions for the algebraic-geometric data of the con-
construction of [21] defining smooth real-valued potential (Л, = 0)
operators H~H0 were given. Novikov formulated the conjecture that
the corresponding potentials constitute a dense family among all
smooth periodic potentials u(x,y).
The main aim of the last section of the paper is to prove Novikov's
conjecture. Again, as in the proof of the theorem on approximation in
Section 2, we need detailed information about the structure of the
Riemann surface for the Bloch functions of the operator #0 cor-
corresponding to a fixed energy level Eo. (The existence of such a Riemann
surface was proved in [20].) From the strictly technical and formal side,
the construction of formal Bloch solutions of the equation Ноф = Еоф
differs significantly from the construction of the Bloch solutions of the
equation Мф = 0, where M is an operator of the form G). Nevertheless,
the most important fundamental features of the construction of the
spectral theory of the operators G) and A1) are entirely parallel to each
other. Because of this, the author hopes that the approach worked out
is applicable to the construction of the spectral theory of arbitrary two-
dimensional periodic differential operators.
Equations of the Lax type constitute a special case of equations of
the KP type. Therefore, the scheme proposed for constructing the

8 l.M. KRICHEVER
theory of perturbations is also applicable in the case of Equation D).
The presentation of the contents of the article starts with the theory of
perturbations of periodic solutions of the KdV and Sine-Gordon
equations. Once more, we stress the fact that even though this
presentation will be independent of the subsequent theory of two-
dimensional equations, it was the KP equation that served as an
example by means of which the basic ideas of the scheme were
formulated. Only after that were the ideas carried over to the one-
dimensional case.
1. PERTURBATION THEORY OF FINITE-ZONE SOLUTIONS
OF EVOLUTION LAX-TYPE EQUATIONS
1.1. General Scheme
Before proceeding to the analysis of specific examples, we shall try to
give a schematic presentation of the basic ideas of the approach
proposed.
As already mentioned in the Introduction, the origin of Riemann
surfaces in the spectral theory of ordinary linear periodic operators
appears now to be self-evident. Indeed, for any such operator L the
space of solutions of the equation
Ly = Ey A3)
is finite-dimensional. Here E is an arbitrary complex number. The
monodromy operator t: y(x) -» y(x+ T) transforms the space into
itself, i.e. it induces a finite-dimensional linear operator f{E) on the
space such that each of the matrix elements of T(E) in the standard
basis is an entire function of E. It follows that the characteristic
equation
R(w,E) = det(w.l-fCE)) = 0
defines a Riemann surface Г such that each point of the surface can be
represented as a pair Q = (w, E) for which there is a function ф(х, Q)
that satisfies the equalities
Ьф(х, Q) = Еф(х, Q), ф(х+ T, Q) = и^(лг, Q). A4)
Such functions are called Bloch functions. In a general situation one

PERTURBATION THEORY 9
can assume that to each point QeF there corresponds a Bloch
function, which is unique up to the multiplication on constant
functions. Note also that for each point Q in general position there is a
unique Bloch solution of the formally adjoint equation, i.e., there is a
row vector ф+(х, Q) such that
фЧх, Q)L = Еф+(х, Q), ф+(х+ T, Q) = п~1ф+(х, Q). A5)
(The right action of operators is defined in the standard way,
ф*щд1я = (-l)i3^+tvi).) A6)
Operators such that the corresponding Riemann surface is of finite
genus are called finite-zone operators. We denote by Mfl the set of all
operators of order n with (/x/) - matrix coefficients such that the
spectral curve Г is of genus g.
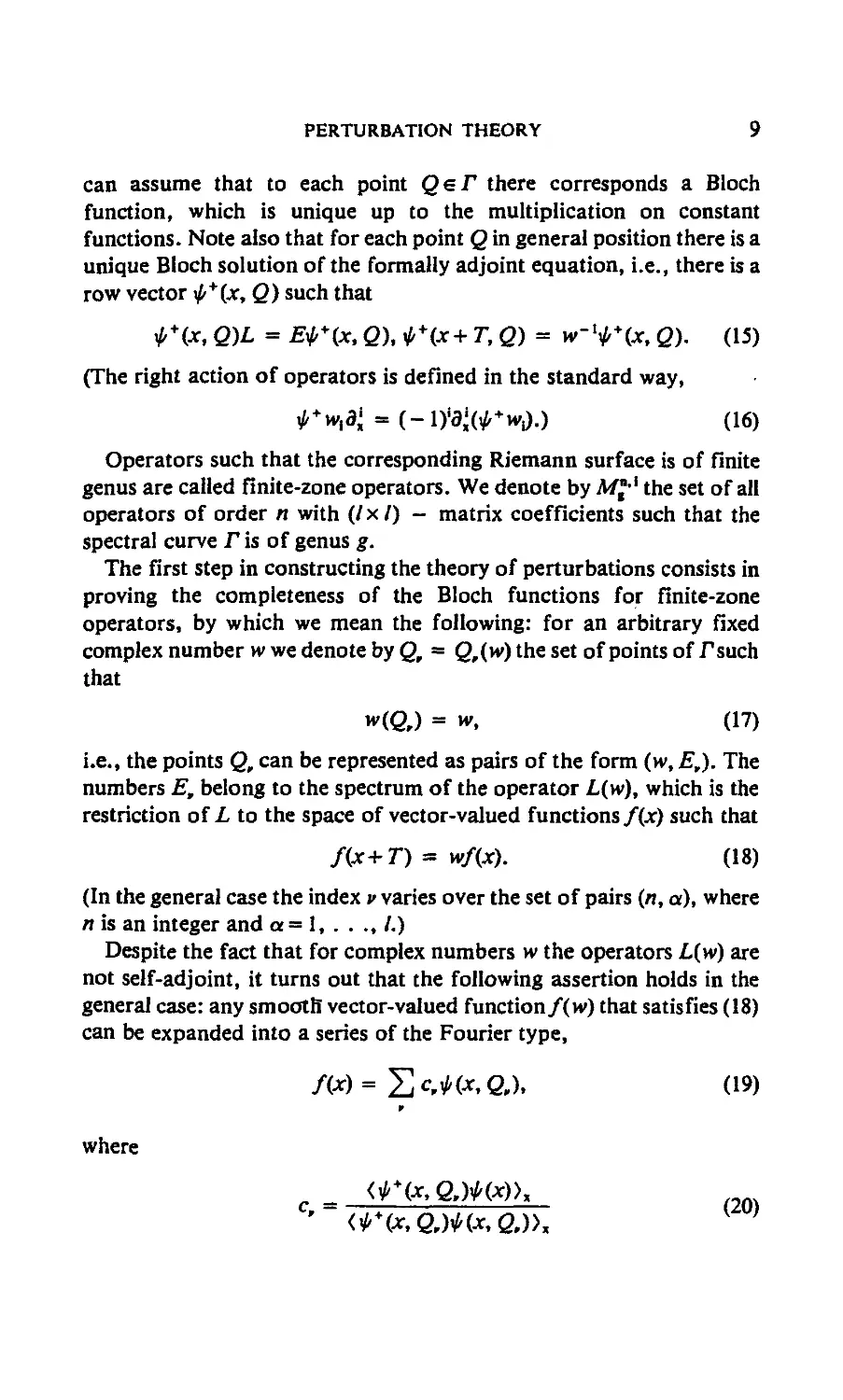
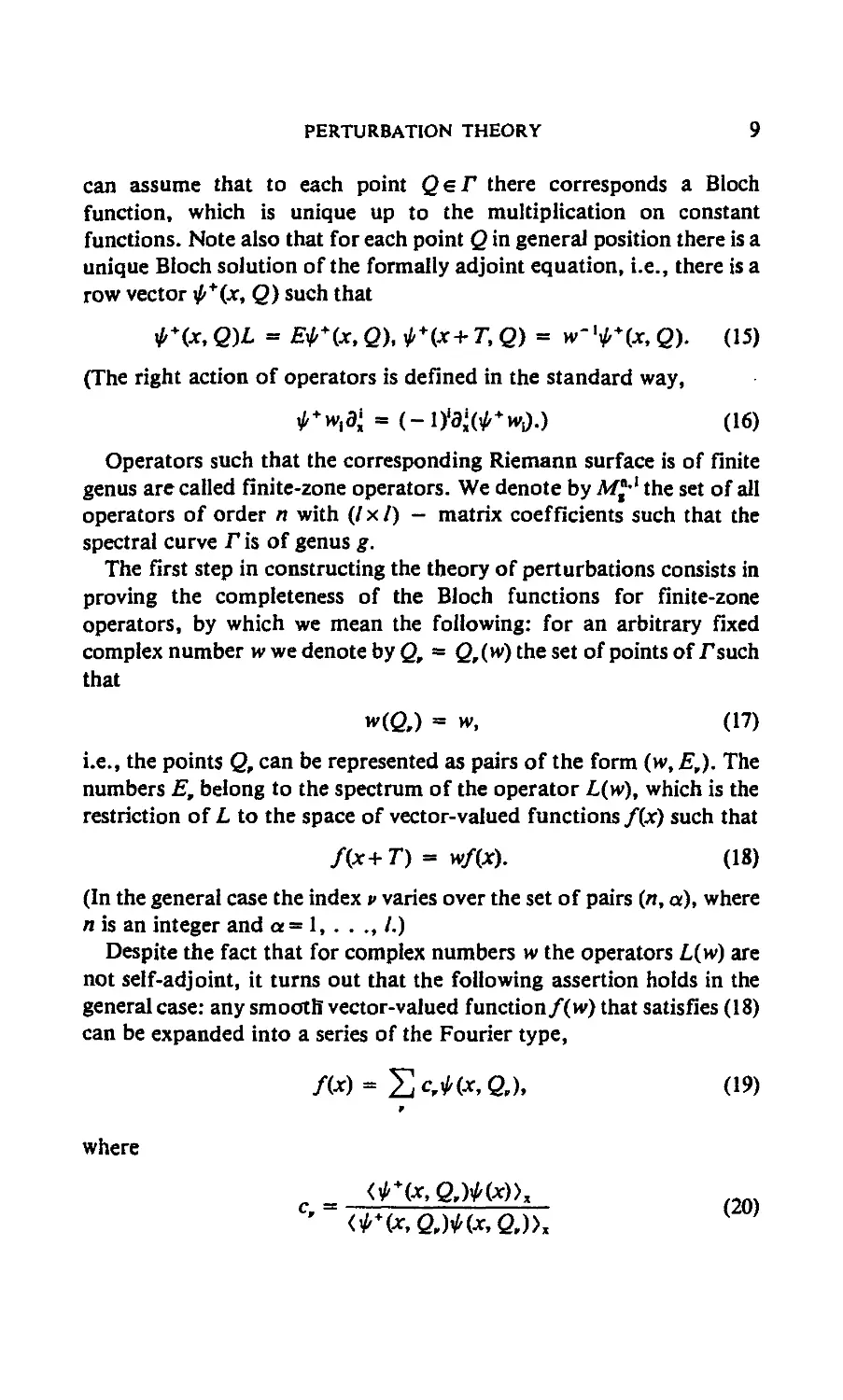
The first step in constructing the theory of perturbations consists in
proving the completeness of the Bloch functions for finite-zone
operators, by which we mean the following: for an arbitrary fixed
complex number w we denote by Q, = Q,(w) the set of points of Fsuch
that
w(Q,) = w, A7)
i.e., the points Q, can be represented as pairs of the form (w, E,). The
numbers E, belong to the spectrum of the operator L(w), which is the
restriction of L to the space of vector-valued functions /(jc) such that
f(x+T)=wf(x). A8)
(In the general case the index v varies over the set of pairs (л, a), where
n is an integer and a = 1, ...,/.)
Despite the fact that for complex numbers w the operators L(w) are
not self-adjoint, it turns out that the following assertion holds in the
general case: any smooth" vector-valued function/(tv) that satisfies A8)
can be expanded into a series of the Fourier type,
f(x)= I]c^(x,e,), A9)
P
where
<*4x, Q,)Hx))x
' <V(xQM{xQ)> K '

8 l.M. KRICHEVER
theory of perturbations is also applicable in the case of Equation D).
The presentation of the contents of the article starts with the theory of
perturbations of periodic solutions of the KdV and Sine-Gordon
equations. Once more, we stress the fact that even though this
presentation will be independent of the subsequent theory of two-
dimensional equations, it was the KP equation that served as an
example by means of which the basic ideas of the scheme were
formulated. Only after that were the ideas carried over to the one-
dimensional case.
1. PERTURBATION THEORY OF FINITE-ZONE SOLUTIONS
OF EVOLUTION LAX-TYPE EQUATIONS
J.I. General Scheme
Before proceeding to the analysis of specific examples, we shall try to
give a schematic presentation of the basic ideas of the approach
proposed.
As already mentioned in the Introduction, the origin of Riemann
surfaces in the spectral theory of ordinary linear periodic operators
appears now to be self-evident. Indeed, for any such operator L the
space of solutions of the equation
Ly - Ey A3)
is finite-dimensional. Неге Е is an arbitrary complex number. The
monodromy operator f: y(x) -* y(x+T) transforms the space into
itself, i.e. it induces a finite-dimensional linear operator f(E) on the
space such that each of the matrix elements of f{E) in the standard
basis is an entire function of E. It follows that the characteristic
equation
R{w,E) = det(w.l-f(£)) = 0
defines a Riemann surface Г such that each point of the surface can be
represented as a pair Q = (w, E) for which there is a function ф(х, Q)
that satisfies the equalities
, Q) = Еф(х, Q), ф(х+ T, Q) = и^(дг, Q). A4)
Such functions are called Bloch functions. In a general situation one

PERTURBATION THEORY 9
can assume that to each point QeF there corresponds a Bloch
function, which is unique up to the multiplication on constant
functions. Note also that for each point Q in general position there is a
unique Bloch solution of the formally adjoint equation, i.e., there is a
row vector ф*(х, (?) such that
ф+(х, Q)L = Еф+(х, Q), ф+(х+ T, Q) = w' 'ф+(х, Q). A5)
(The right action of operators is defined in the standard way,
Operators such that the corresponding Riemann surface is of finite
genus are called finite-zone operators. We denote by M*-1 the set of all
operators of order л with (/ x /) - matrix coefficients such that the
spectral curve Г is of genus g.
The first step in constructing the theory of perturbations consists in
proving the completeness of the Bloch functions for finite-zone
operators, by which we mean the following: for an arbitrary fixed
complex number w we denote by Q, = Q,(w) the set of points of Tsuch
that
w(Q,) - w, A7)
i.e., the points (?, can be represented as pairs of the form (w, E,). The
numbers E, belong to the spectrum of the operator L(w), which is the
restriction of L to the space of vector-valued functions/(r) such that
f(x+ T) = wf{x). A8)
(In the general case the index v varies over the set of pairs (л, a), where
л is an integer and a = 1, ...,/.)
Despite the fact that for complex numbers w the operators L(w) are
not self-adjoint, it turns out that the following assertion holds in the
general case: any smooth" vector-valued function/(tv) that satisfies A8)
can be expanded into a series of the Fourier type,
/M - 2 *„*(*, Q.), A9)
where

10 l.M. KRICHEVER
are the coefficients of the series. (Here and in what follows < •>„ denotes
the mean value with respect to x.)
The method of contour integrals is used in the proofs of similar
assertions given in subsequent sections.
Relations A9) and B0) enable one to make without difficulty the
next step in our scheme, namely, to construct the perturbation series
for a Bloch solution of the perturbed operator L + 8L.
What is it needed for? Being able to construct the solutions of a non-
nonlinear equation depending on parameters, one can easily obtain the
solutions of the linearized equation. To do this, one only needs to dif-
differentiate the solutions of the non-linear equation with respect to the
parameters.
Among such solutions of the linearized Equation D) with an under-
underlying finite-zone operator Lo corresponding to a curve of genus g there
is a subspace that corresponds to the subspace tangent to MSD>I. It is also
possible to construct solutions corresponding to 'transversal'
variations.
The study of the first order of the perturbation theory of the Bloch
solutions for the operator L0 + 8L enables us to prove that we can
thereby find a full system of solutions of the linearized Lax type
Equation D).
We present very schematically the idea of such a proof (as well as all
other ideas in this section). It follows from A9) and B0) that the Bloch
solutions of the equation
, Qo) = (£0+5£)i?(jc, Qo) B1)
are given by the standard formulae
B2)
up to a second-order term. There are singular terms in B2).for those Qo
for which there is a point Q, such that E, = E{Qr) = E(Q0) for v Ф 0.
The condition ensuring that there are no such singularities, which
means that the equalities
2o)>x - 0 B3)

PERTURBATION THEORY 11
if
E, = £0( v Ф 0,
hold for all 'resonance' pairs {Q,, (?„), enables one to prove after
analysing B2) more carefully that the series converges on Г to a
function which is meromorphic everywhere except at £=».
Moreover, one can supplement B3) by N = dim М^л additional
conditions of the same type, so that the conditions combined with B3)
imply that the series B2) defines a function ф~(х, <2„) on Г equipped with
the same analytic properties as ^C*iQo)- Since the Baker-Akhiezer
function is unique, it follows that 4>(xxQ) = 4*(x,Q), which means
that 8L = 0. (Looking ahead, we remark that B3) is equivalent to the
fact that 8L belongs to the space tangent to M,"*1.) Half of the condi-
conditions mentioned above but not written down can be expressed in the
form
= 0. B4)
Combined with B3), these conditions mean that SL is a linear 'iso-
spectraT deformation, i.e., a deformation which does not change Г. It
is known that such deformations, which are tangent to the 'Jacobian',
coincide with the flows of 'higher-order equations' associated with D).
Apart from proving the completeness of the constructed solutions of
the linearized equation of the Lax type, the approach makes it possible
to construct a biorthogonal basis of solutions of the adjoint equation.
If we interpret the space for the linearized system as being the space
of periodic operators 6L of order л - 1 (л = deg L), then any pair g*(x),
f(x), where g* and/are a row vector and a column vector such that the
equalities
f(x+ T) = w/Cr), g+(x+ T) = w-lg+(x) B5)
hold, correctly defines an element of the adjoint space by the formula
B6)
In this interpretation it turns out that the pairs {ф+(х, t, Q,), ф(х, t,
Qo)} and {ф*(х, t, Q), ф(х, t, Q)} supplemented by a finite number of
elements form a basis of solutions of the adjoint equation cor-
corresponding to the linearized Equation D).
The fact that each of the pairs is a solution of the adjoint equation is
extremely general.
Indeed, let ф* and ф be solutions of the system

12 I.M. KRICHEVER
= О,
B7)
that satisfy B5) for some w (which means that the mean value <->x in
the formulae below makes sense). Then for any solution 6L of the
linearized Equation D)
6LX = 16A,L] + [A,bL\ B8)
the equality
dt(W6Lt>J = 0 B9)
holds. The proof of B9) is elementary:
= <,ф?5АЕф>х - {ф!ЕЬАф)х = 0.
Equality B9) is equivalent to the fact that {ф*, ф) is a solution of the
adjoint equation to B9).
The proofs of the biorthogonality relations for the systems of
solutions of the linearized and the adjoint equations, which will be
constructed, are not as elementary as B9) and require the scheme which
was briefly presented above.
Note: a pair of Bloch solutions ф? and ф of equation B7) is a
solution of the formally adjoint equation corresponding to equation
B8) even if their Bloch multipliers w, and w do not satisfy the relation
w, w = 1. To prove that this is the case, we note that equation B8) can
be restricted to the space of 8L and 8A such that 8L(x+ T) =
(w, w)~16L(x) and 8A(x+T) = (и^и^'б/Цл), and the previous
discussion can be repeated.
To conclude the present section let us note that equations of the Lax
type D), with L and Л being differential operators of the form B), yield
only a part of the set of spatially one-dimensional integrable evolution
equations. In a more general scheme (for example, for the S&ne-Gordon
equation), the operator L contains a rational function of the spectral
parameter £". Of course, in this case formulae A9)-B2) are of different
form. But it is only the formulae that change, not the essence of what is
happening.

PERTURBATION THEORY 13
1.2. The Korteweg-de Vries Equation
The theory of perturbations of periodic finite-zone solutions of the
KdV equation was considered in [24], where the completeness of the
constructed system of solutions of the linearized KdV equation was
proved and the biorthogonal basis of solutions of the adjoint equation
was found. The idea of [24] was different from that of the scheme just
presented.
The 'л-zone' solutions of the KdV equation were first defined [25] as
the extremal points of the functional
5tf = 0, H = /n+2 - S ck/k> C0)
where /k[u] is an infinite family of integrals of the KdV equation in
involution.
The proofs of the assertions stated are followed by considering the
'Hessian' of the functional H, i.e. the second variation operator for H
in a neighbourhood of an extremum. It turns out that the solutions of
the linearized equation constructed with the aid of 'variations' of
finite-zone solutions 'diagonalize' the quadratic form 62H.
Below we shall obtain the same assertions as in [24] using different
methods. We shall analyse the case of the KdV equation as an example
of the general scheme.
We recall briefly the information concerning the spectral theory of
d2
finite-zone Sturm-Liouville operators L = -—3 + uo(x).
If uo(x) is a solution of C0), then the spectrum of the operator L
considered on the entire axis contains no more than n gaps [Еъ, £"а+|],
i— 1, . . ., n. The end-points Ex of the zones are simple points of the
spectrum of the periodic and the antiperiodic problem for L.
The Riemann surface Г for the Bloch functions is a hyperelliptic
curve defined by the equation
The Bloch function ф(х, Q) with Q = (у, Е) е Г is uniquely defined by
the following analytic properties:

14 I.M. KRICHEVER
(a) the function is meromorphic everywhere except at the 'infinitely
distant' point Po (£=») and has one pole 7, on each of the
cycles over the 'forbidden zones' £E"a, £"a+l]
(b) in a neighbourhood of Po the function has the form
1>Qc. Q) = Wl + E «*)*■'), C2)
For any sequence of (Eit 7})) there is a unique function ф(х, Q) with
the given properties such that
(- д2% + щ(х))ф(х, Q) = E(QW(x, Q), C3)
where
uo(x) = 2/{|ж(х). C4)
In the standard way we define a normalized basis of hoiomorphic
differentials ык on Г, the matrix 5Ш of their 6-periods, and the cor-
corresponding Riemann theta function 9@„ . . ., 0a). (For more details,
see [4, 5], where the proofs of the formulae given below can also be
found. In a more general case the proofs will be given in part 1 of
Section 2; see also [2, 3].)
The Baker-Akhiezer function ф(х, Q) is of the form
Here A(Q) is the Abel function:
Q
Ak(Q) = J «k- C6)
OB
The differential of the quasimomentum
2л+1
dp = -^- d£, Л = TT (£"-£"i) C7)
VrW) t.i
is uniquely normalized by the conditions

PERTURBATION THEORY 15
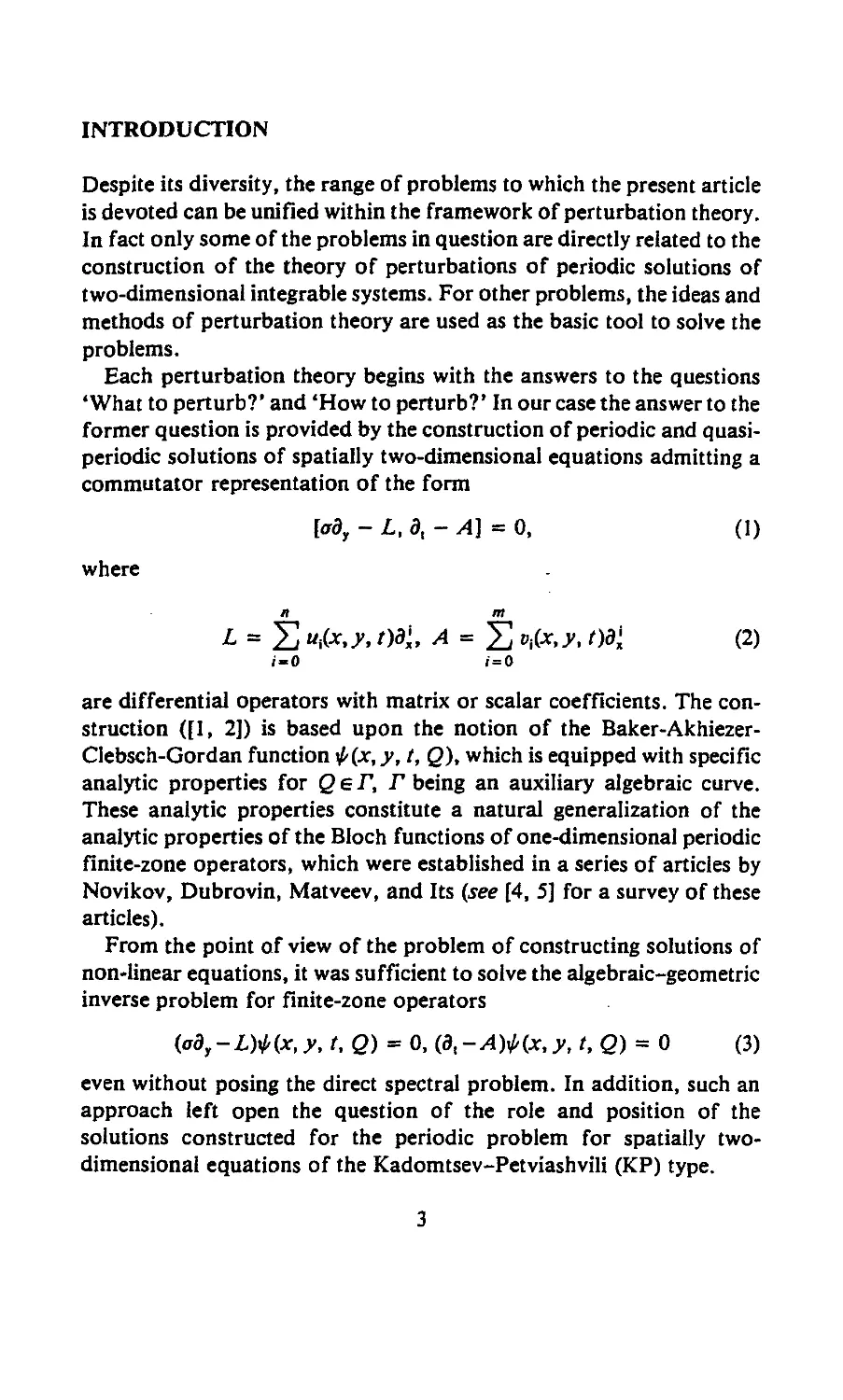
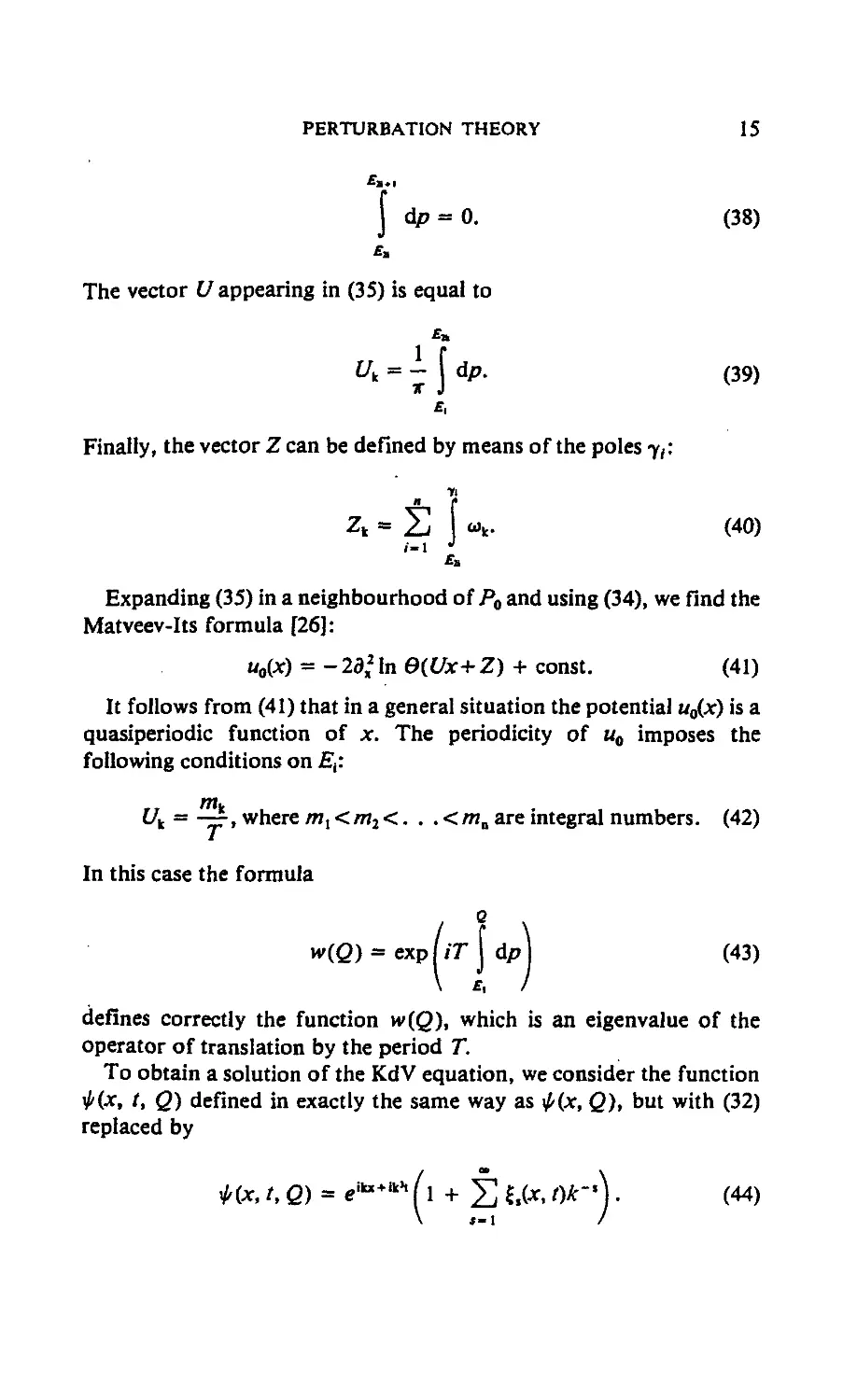
dp = 0. C8)
The vector U appearing in C5) is equal to
Uk = т 1 dp' C9)
Finally, the vector Z can be defined by means of the poles 7,:
я f>
Z, = S «*• D0)
1-1 J
Expanding C5) in a neighbourhood of Po and using C4), we find the
Matveev-Its formula [26]:
Щ(х) = -2Э,2 In 0(C/x+Z) + const. D1)
It follows from D1) that in a general situation the potential uo(x) is a
quasiperiodic function of x. The periodicity of u0 imposes the
following conditions on Et:
Uk = —г, where m, < m2 <. . . < /nD are integral numbers. D2)
In this case the formula
iT ] dp]
= exp[iT I dp I D3)
defines correctly the function w(Q), which is an eigenvalue of the
operator of translation by the period T.
To obtain a solution of the KdV equation, we consider the function
^(*t t, Q) defined in exactly the same way as ф(х, Q), but with C2)
replaced by
Их, t, Q) = <?!к*+ил (l + S «г, О*") • D4)

16 I.M. KRICHEVER
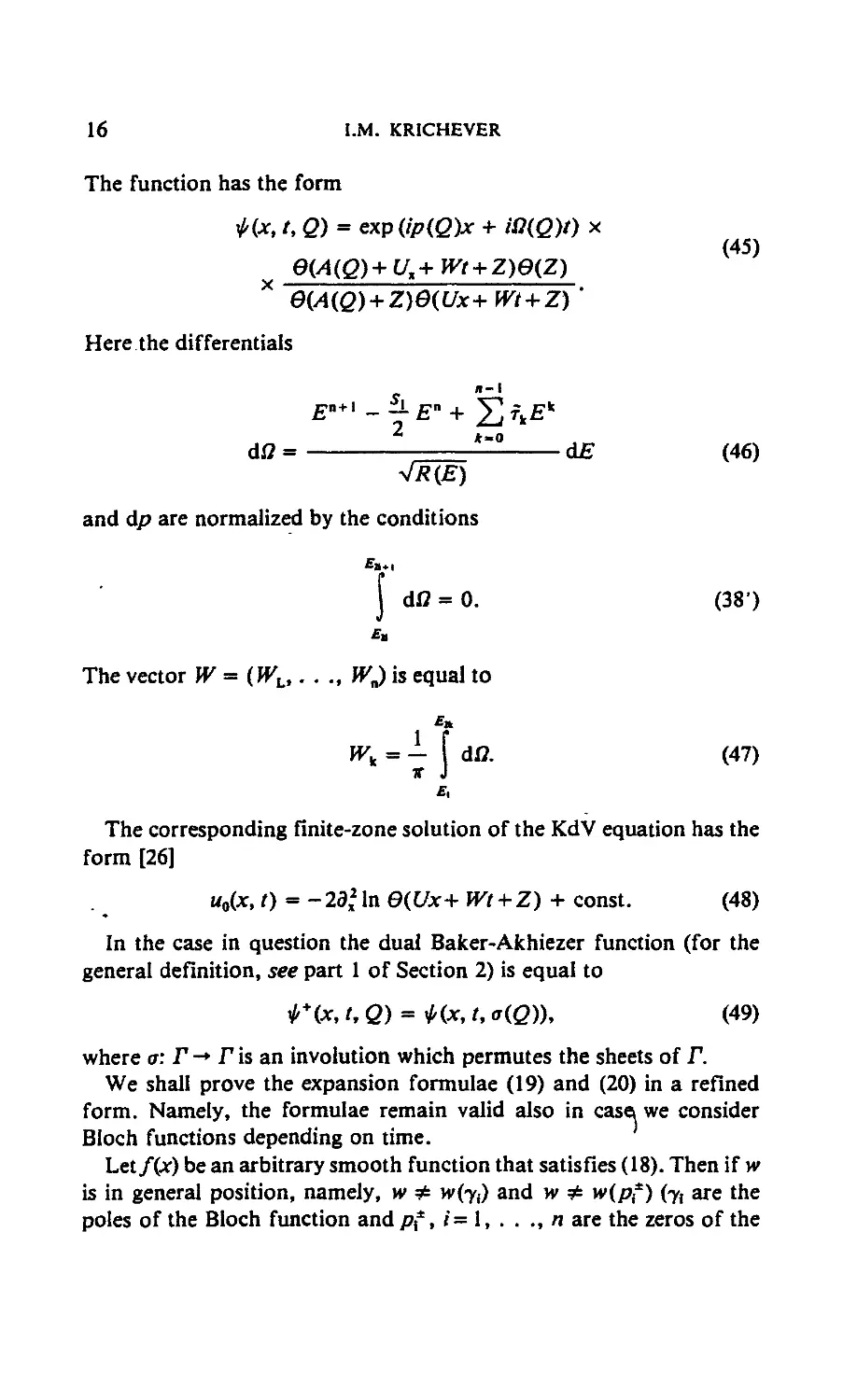
The function has the form
Ф(х, t, Q) = exp(ip(Q)x + iQ(Q)t) x
D5)
9{A(Q) +Ux+Wt + ZN(Z)
6(A{Q) + ZN(Ux+ Wt + Z) '
Here the differentials
dfl = *"" dE D6)
and dp are normalized by the conditions
dO = 0. C8')
The vector W = (WL,. . ., Wn) is equal to
Wk - i J dfl. D7)
The corresponding finite-zone solution of the KdV equation has the
form [26]
Щ(х, t) = - 2Ъ\ In 0(С/л:+ »7 + Z) + const. D8)
In the case in question the dual Baker-Akhiezer function (for the
general definition, see part 1 of Section 2) is equal to
*4x,t,Q) = Ux,t,o{Q)), D9)
where а: Г-* Г is an involution which permutes the sheets of Г.
We shall prove the expansion formulae A9) and B0) in a refined
form. Namely, the formulae remain valid also in casa we consider
Bloch functions depending on time.
Let/(jr) be an arbitrary smooth function that satisfies A8). Then if w
is in general position, namely, w Ф yv(y{) and w Ф w(p?) {yx are the
poles of the Bloch function and p*, / = 1, . . ., n are the zeros of the

PERTURBATION THEORY 17
differential dp от, equivalently, the zeros of the function
<Ф+(х, t, Q)Hx, t, G)>x), we have
х, t), E0)
n
where фп = ф(х, t, G»), ф: = ф+(х, t, Qa), and
Note. The restrictions imposed on w are not very strict. One can
easily see from the following proof that the series in E0) can be
continuously defined also for the excluded values of w.
To prove E1), we consider the integral
с о
where С is a contour containing the infinitely distant point, and where
dfiis the following differential on Г:
This differential has an important property. Namely, it is holomorphic
on Г except at the point Po (£■= <»), which is equivalent to the fact that
the zeros pf of dp coincide with the zeros of the function (ф(х, t,
п)Ф*(х, t, Q))x. (For the proof of this assertion in a more general
situation, see [27J or part 1 of Section 2.) It follows from E3) that the
zeros of dS coincide with the poles of ф and ф *. Hence,
П №-7.)
jT dO = ' d£. E4)
y[R(EJ
(In cases where it does not lead to any misunderstandings we shall use
the same notation for the points у-,еГ themselves and for their
projections onto the £"-plane.)
By virtue of what is said above, the integrand has poles at the points
Qn — Gn(w) only, and the residues at these points coincide with the
terms of the series E0).

18 I.M. KRICHEVER
Therefore, Sc is equal to a partial sum of the series E1). On the other
hand, choosing p (wherep = к + O(k~') is the quasimomentum) as
the local parameter in a neighbourhood of infinity, we find from D4)
that
x-x')dx'd|'
")>- <55)
с О
Thus, letting с -+ <x, we find that Sc tends to the sum of the Fourier
series, i.e., it tends to/te).
Let v(x) be a smooth periodic function such that o(x + T) = v{x).
Lemma I For any non-resonance point Qo e Г such that Qo Ф yjt
pf, there is a unique constant e(t) such that the equation
, О-Ео)ф(х, t, Qo) = ь(х)ф{х, t, Qo) + еA)ф(х, t, Qo)
E6)
has a unique solution which satisfies the conditions
ф(х + T, t, Qo) = wo<H*. t. Go), w0 = w(Q0)
Proof From E0) it follows that for any point Qo in general
position, i.e. for any non-resonance point such that w(Q0) ф wGj),
w(pf), can be represented in the form
Ф(х, t, Go) = S сп«)фп(х, t), E8)
ff#0
фп(х, t) = ф(х, t, QD).
Substituting E8) into E6), we get
, t,Q)~ 2L
_
By analogy with what is done to investigate the series in Section
2.5.14 one can prove that E9) converges and admits analytic
continuation to all non-resonance points Go except Go=7j. Pf-

PERTURBATION THEORY 19
Before proceeding further, let us explain the meaning of resonance
points. A pair of points Q and Q' is a resonance pair if E(Q)=E(Q')
and w(Q) = w(Q'). The former equality means that Q' = a(Q), i.e. the
points of the pair lie on two sheets of Г over the same point on the E-
plane. Since w(o(Q)) = w~*(Q), the resonance points are such that
w\Q)=l (except for the end-points of the zones). All of them are
double points of the spectrum of the periodic or the antiperiodic
problem for L and can be found from the equation
«.*
— I dp = ± —, where тФтк, . . ., mn are integral numbers F0)
т J T
E,
(the numbers mk are defined in C8) and D2)).
As explained at the end of part 1, each of the functions
#*«, = Их, t, е*)ф+(х, t, el) = фЧх, t, el) F1)
is a solution of the equation
Ф1-Aд2%-±и0)дхФ = 0 F2)
adjoint to the linearized Korteweg-de Vries equation
O. F3)
Ф(х, t, Q) ф*(х, t, Q) is also a solution of this equation. It is important
to note that among the last functions constructed there are only n+l
linearly independent ones. Indeed, according to the definition of ф+,
the function ф(х, t, QW*(x, t, Q) assumes the same values on both of
the sheets of Г and is regular at infinity. Consequently, it is a rational
function of E and has the form
Й да-»)
As the functions forming a basic family we can choose
Фо m 1, #„ = ф(х, t, Е2к)ф+(х, t, En). F5)
(We recall that тФти . . ., mB in F1).)
Let yk(t) e [£■», £2k+,] be the zeros of ^@, /, Q) Cyk(O) = yk). We have

20 I.M. KR1CHEVER
,Q) Tk(x,t;t0)
O{\) F6)
in a neighbourhood of y\(t0).
We denote by Ф-„^(х, t) the function that is equal to
F7)
for 0 < x < T, and is extended by continuity to the remaining values of
x. Since тк@, t; t) = тк(Т, t; t) = 0, it follows that #_„, is continuous
with respect to x, but its derivatives have discontinuities at x=nT.
We mention that by virtue of the Note at the end of part 1, т£(х, t; tj
satisfies Equation F3).
Theorem 1 For any t, the functions Ф„(х, t), where meZ, form a
minimal basis in L2(S1).
Proof Let v(x) be an arbitrary smooth periodic function that
satisfies the conditions
(и(х)Фт(х,фх = 0 F8)
for all m. We claim that v{x) ■ 0. To prove this identity, we consider
the series E9). Simple estimates show that the series is holomorphic
everywhere except at the resonance points e* and the points Po = <», y.t
aiidp*. Condition F8) with m Ф±т1, . . ., ±ma means that ф does
not have a pole at e*.
We consider the behaviour of ф in a neighbourhood ofpf. In such a
neighbourhood all but one of the terms of the series E9) are regular.
The singular term corresponds to the point Q^ that tends to p£ as
Go - A*. Therefore, £„„-£0-0 and <^; >x - 0 as Qo - /tf. For
m= ±/л„ . . ., ±/п„, conditions F8) are equivalent to the fact that
Ф (x, t, Qo) has no second-order poles at p£.
This can be rewritten as a regularity condition for the function
, t, Go) - Ф(x, t, Go) = *?' °: ^\ Ф(x, t, Go) F9)
Ф(°Q) )
at the points /?jf.
Now, we consider the last group of conditions, which follow from
the requirement that ф(х, t, Qo) be regular at 7,@-
We shall evaluate the function Фту(х, t) defined by the series

PERTURBATION THEORY 21
Неге бо=7к(О» Фш-Их, t, GB), and w(QJ = wGk@) = wk(').
We consider the contour integral
Since E = p2 + o(l),
) G2)
in a neighbourhood of infinity. This means that if 0 < x < T, then St
tends to zero as с -* <». The residues of the integrand at the points
Qn ф yk(t) are identical to the terms of the series G0). Apart from the
points Qn with n Ф 0, the integrand has a second-order pole at Qo -
7k(/) and a first-order pole at yk (t) = a(yk(t)).
Computing the residues of the integrand at these points (and using
the fact that ф(о, t, yk(t)) = 0), we find that
Фп» = сф2(х, t, yk(t)) + Ьф(х, t, yk(t)№+(x, t, yk(t)). G3)
'■'. Consequently, from conditions F8) it follows for | m | = m,,. . ., /rtB
that ф(о, t, yk@) = 0. Thus, ф(х, t, Q) is regular everywhere except at
infinity and at the points yk = 7k@), where it has at most simple poles.
In a neighbourhood of infinity
' Ф(х, t, Q) = eikx+'kHO(k~l). G4)
By the uniqueness of the Baker-Akhiezer function, we find that
Ф(х, t, Q) = 0. Thus, in accordance with Lemma 1, we find that if u(x)
satisfies F8), then the right-hand side of E6) vanishes.
-W.'.e> = 0. G5)
, t, C)^(x, /, Q)\
The second term is equal to zero by virtue of F8). Therefore v(x) ■ 0,
and the completeness of the family of functions Фт(х, t) is proved for
all f.
To prove that the functions Фт(х, t) form a minimal basis, we
construct the adjoint system of functions.

22 I.M. KRICHEVER
As already mentioned, for any sequence of end-points Ex
of the zones, the corresponding solution of the KdV equation given by
D8) is a quasiperiodic function of x. The condition of periodicity of
щ(х, t) with respect to x is equivalent to n transcendental equations
for Е-,.
If we restrict ourselves to those KdV solutions that satisfy the
equality
<м(лг,0>х = 0, G6)
then Ел, /'= 1, . . ., л are the independent parameters. Thus, uo(x, t)
depends on the quantities Еъ and y-, playing the role of parameters:
uo(x,t) = щ(х, 1\ЕЪ, 7l).
We define the functions
вш'ш
Here 0 < /n, < . . . < mn is a sequence of numbers defined by D2).
The functions given by G7) form a basis, which is tangent to the inter-
intersection of Ml with the space of functions satisfying G6).
We shall find the basis of 'transversal' directions. To do so, we
consider the hyperelliptic curve Ftm obtained by opening a small zone
in place of the point em. The curve is given by the equation
2л+1
У = ((£-О2-е2)П №-5)- G8)
i-1
According to D8), the curve defines a solution ut(x, t) of the KdV
equation. The derivative —- \ is a solution of the linearized KdV
& J.-o
equation.
Let us give the necessary information about holomorphic dif-
differentials on Г-т. Let <j, . . ., шв+1 be a basis of normalized dif-
differentials on P#m. If U|, . . ., <■>, is a basis of normalized holomorphic
differentials on Г and a>* is a normalized differential of the third kind
on Г with residues ± 1/2т/ at the points e*, then
|u,-<3,| < O(«2), 1=1 n, |<5n + 1-<2*| < O(e2) G9)

PERTURBATION THEORY 23
outside small neighbourhoods of these points. Let £'•" and В be the
matrices formed by the periods of Г*-1" and Г, respectively. Then it
follows from G9) that
w* = Л,(О - А-,(е~) = 2АГ. (81)
ь,
(The latter follows from Riemann's bilinear relations.) For the matrix
element ^V+i.n+i we nave
£»?..»+1 = —- On « + rm + O(e2)). (82)
The theta function 9 = 9{z{,. . ., zn+,) constructed from £'•" is equal
to
ё= 9(z) + ee'-[9(z + 2>r)e2A"' - 9{z-2Am)e'lwil">] + O(e2),
(83)
where z = (г, г„) and A" = ИГ), with A? defined by (82).
We consider the finite-zone potential п(х, t) corresponding to the
curve Г'1™ and to the divisor of poles 7,, . . ., yn+l. The potential is
defined by formula D8) with ё substituted for the theta function. The
vectors formed by the 6-periods of the differentials dp and d6 on Г*-т
are equal to
0; = Ut + O(e2), Щ = Щ + O(e2), /= 1 n,
(84)
From D8) and (83) we find that
8u = й - и
(85)
where the functions v±m, where m > 0, are given by the formulae
() (x )
v±m = _1 1 Э2 _1J 1 e t . (g6)

24 i.M. KRICHEVER
The normalization of vm(x, t) is chosen so as to ensure that equalities
(87) are satisfied. Since the linear part (85) defines the solutions of the
linearized KdV equation F2) for any za+i, it follows that each of the
functions v±m is a solution of F2).
Lemma2 The functions vm,meZsatisfy the relations
<#ш(дг,ОМ*.О>,-**.-. (87)
The proof of relations (87) (and of similar relations discussed later) is
based on computing the singularities of the derivative фт = дтф(х, t, Q)
for certain variations r of Г. We have
(88)
where ф(х, t,p) is given by E9) with v(x, t) = uT(x, t\r). Note that
formula (88) holds if the derivative with respect to r with constant
p=p(Q) is taken. The derivative with constant E is equal to
АФ do
фг(х, t,E) = фг(.х, t,p) + dTE-£ ^|,
(89)
дЕ( <ф+(х,!,р)у(х,{)ф(х,1,р))х
т (Р} <ф+(х,(,р)ф(х,1,р>х '
Since we know the singularities of фт, we can compare them with those
of ф to get (87). As an example, let us analyse one of the typical
relations for m' Ф mt, . . ., mn, since this case involves the least
elementary computations.
To compute д,ф with the variation Г*>т which corresponds to opening
a zone in place of em, we use D5) and formula (83) for the variation of
the theta function. д,ф acquires a singularity only as Q -» e* and only
due to the fact that the (л + l)-st component of A{Q) is equal to
q \
Aa+i(Q) = J "m = 2^1n(£(Q)-eJ + rm + O(e) (90)
to within an accuracy of order O(«). It follows from D5) and (83) that

9(A(Q) +
PERTURBATION THEORY 25
e'- Г
tVU'° E(Q)-em[
6(Z)-
Wt + Z)
»)-2)-
+ 0A).
Let us now find the singularity of ф(х, t, E) at e*. We have (since
Comparing (88) with (87), we find that
<Фа(х,0иа(х,фх=1. (93)
Since \j/t is regular everywhere except at em, it follows that < *m, pm > =0
if m Ф m'.
In a similar way one can analyse the case of variations of the end-
points £, of the zones and the poles yx. The lemma is proved. The
assertion of the lemma shows that the functions Фт form a minimal
basis.
Theorem 2 follows immediately from Theorem I and Lemma 1.
Theorem 2 Each of the functions vm(x, t), where meZ, defined by
G7) and (86) is a solution of the linearized KdV Equation F2), and for
each t the functions form a minimal basis in %\Sl).
As already mentioned in the Introduction, having found the basis of
solutions of the linearized KdV equation and the adjoint system of
functions, we can easily write down an asymptotic solution of the form
(9). However, as shown in [28, 29], since there are resonances for
л > 1, the uniform estimate of the leading correction term for uo(x, t)
turns out to be of the form O(e1/2) rather than O(e). (In this connection
note that the assertion of Theorem 4 of [24] only holds for /i = I.)
Let us also mention papers [30] and [31], in which the results of
[24] were used to analyse, by means of the KdV equation, taken as an
example, whether the Kolmogorov-Arnold-Mozer theory can be

26 I.M. KRICHEVER
generalized to infinite-dimensional systems.
To conclude part 2, let us consider a typical question, which is funda-
fundamental for the construction of the KAM theory, namely, that
concerning the non-degeneracy of the 'frequencies' as functions of
action-type variables.
By virtue of C9) and D0), for each hyperelliptic curve Г of genus n
there is an associated pair of л-dimensional vectors [/and W. (Hence-
(Henceforth, as everywhere in this part, we consider only hyperelliptic curves
with real branching points: £,<...< E2a+I.) To any such curve Г
there correspond finite-zone solutions. Let /, = <мо> be the mean
values of these solutions with respect to x. (We note that /, depends
only on Г and can be determined from the expansion of quasi-
momentum p in a neighbourhood of infinity,
/> = * + /,*"' + O(k'3), к = >ffi.) (94)
Theorem 3 The Jacobian of the transformation
MS = {£,<...< E^,} -*{U,W, /,} (95)
does not vanish anywhere.
Proof Suppose that there is a variation Г, of the curve Го preserving
the vectors U, W, and the constant /, to within an accuracy О(т2).
The integrals p(Q) and O(Q) of the differentials D7) and D6) are
multivalued functions on each of the curves Гт. At any point in general
position, p can be chosen as the local parameter. Then, locally,
0- Q(p, r). We claim that under the assumptions made,
£ 0{p, t)
o
= 0. (96)
-0
Indeed, since in the first-order approximation the periods of dfi are
independent of r, it follows that d,Q\tm0 is a single-valued mero-
morphic function on Го. The function has poles at the points p£, where
^Ip-Рг, rather than p, are the local parameters. In a neighbpurhood
of infinity we have '
О = A:3 + O(k-1) =p> - 3/,p + O(p~l). (97)
It follows that the singular part of Ois independent of т. Thus, 3f0|r_0
vanishes at infinity. At the branching points Eu and £„+, the quasi-

PERTURBATION THEORY 27
momentum p assumes constant values Uk for all т to within an accuracy
of order O(t2). At the same points I? has constant values Wk to within
О{тг). For£=£, we havep(£,) = QiEt) = 0. It follows that
dTQ\r.o(.Ed = 0, /= 1, . . ., 2л + 1. (98)
This meromorphic function with no more than In zeros vanishes at a
Bл + l)-st point. This is possible only if the function vanishes
identically.
We will expand Q(p, r) in a neighbourhood of the point p*, which is
a zero of the differential dp. By differentiating I? with respect to r, we
find that for the equality dr0= 0 to hold it is necessary that the
condition
dTpk*dl2(pf) = 0, k= 1 л (99)
should be satisfied. Hence, there is at least one common zero of the
differentials dp and dO. Using the same argument as in the proof of
Lemma 9 one can show that for any periodic variation 6u the following
equation holds:
фф+ » = iSp « C£+f и)#* » - | «6ихфф+». A00)
The zeros of the functions < фф * > and < C£+f и )фф * coincide with the
zeros of the differentials dp and dfi, respectively. Therefore, if the
common zero exists then
0 = «дихф(х, 1,рк)ф+(х, Л а)». A01)
But the choice 6ux = фф* leads to contradictions, which proves the
theorem.
1.3. The Sine-Gordon Equation
Finite-zone solutions of the Sine-Gordon equation were first con-
constructed in [32]. (For a presentation of the construction of these solu-
solutions carried out with the aid of the Baker-Akhiezer functions, see
[33].) Applications of averaging methods to multiplicative finite-zone
solutions of the Sine-Gordon equation were developed in [34, 35].
Basically, the articles were concerned with the leading term of the
asymptotic expansion. To evaluate the subsequent corrections, it is
necessary to construct a complete system of solutions of the linearized
equation, which constitutes the main part of the present section.

28 I.M. KRICHEVER
The construction of finite-zone solutions to the Sine-Gordon
equation will be given in a form which differs slightly from that which
is generally adopted (for more details, see [36]).
Let Г be the hyperelliptic curve defined by the equation
A02)
/-i
For any sequence of л points in general position 7,, . . ., 7n there are
unique functions фх(х, t, Q) and ф2(х, t, Q) such that (i) the functions
are meromorphic everywhere except at the points P+(E=0) and
P_(E= 00), and have at most simple poles at the points yk (if the points
are different); and (ii) in neighbourhoods of the points p± the functions
are of the form
-A , k± =
/
, t)k-A , k± =£* A03)
/
and are normalized by the conditions
c,*-cj«l. A04)
It can be proved in a standard way that the vector-valued function
Ф(х, t, Q) with components фг and ф2 satisfies the equation
д.ф = O(x, U Е)ф, д+ф= Их, t, Е}ф, A05)
where
Э* = Тх ± It' A06)
and where the matrix-valued functions О and V are equal to
with eiu = c2"/ci". From the compatibility condition for the system of
linear equations A05) we find that u(x, t) and v(x, t) satisfy the
equations

PERTURBATION THEORY 29
ы = 2 sin w,
which are equivalent to the equation
(Э,2 - dl)u + 4 sin и = 0. A09)
(In what follows, by the Sine-Gordon equation we shall mean system
A08).)
To obtain real-valued solutions it is necessary and sufficient to
restrict oneself to the discussion of real hyperelliptic curves with an
antiholomorphic involution г: Г-* Г such that
A10)
Besides, it is required that the divisor of poles 7,,. . ., ya of the Bloch
function along with the conjugate points 7* = 7G,) form a set of zeros
of the differential of the third kind dC, which is holomorphic every-
everywhere except at the points P± where there are residues which are equal
to ± 1/2. (For more details on the conditions ensuring that finite-zone
solutions to the Sine-Gordon equation are real-valued and regular, see
[37, 38].)
Under the conditions mentioned above, u{x, t) and v(x, t) are real-
valued functions and the row vector
ФЧх, t, Q) = Шх, t, t(Q)), EUx, t, t{Q))) (П1)
satisfies the conjugate system of equations
-д.ф* = ф+О, -д+ф* = ф*У. A12)
We denote by dp the differential of the form
En+1 + a
idp =
uniquely normalized by the conditions
If the integrals of dp along any cycle a are commensurable, i.e.,

30 I.M. KRICHEVER
с„ = —f, where ma are integral numbers A15)
then u(x, 0 is periodic with respect to x.
Considering the functions ф(х, t, Q) and ф*(х, t, Q') and letting
Q' -» Q, we find from A05) and A12) that the equality
1 / . 1 \
/dp ^+^), = — d£ ( Ф\е~тфг — фг—,^i ) A16)
2 \ £ /»
holds. It follows that
,л 'dp _ dE
(For more details on the proofs of similar assertions, see Section 2.1.)
We shall now prove that the system of Bloch functions is complete.
Let wbea complex number in general position, i.e. such that the
equation
w(Q.) = w A18)
has only simple roots Q,. Hence, as before in part 2, the multiplier is
given by the formula
Q
^\ p(Q) = j dp.
A19)
It follows from A15) that w(Q) is correctly defined.
The points Q, represent two sequences converging to the points P±,
respectively: Q, = {QB>±, n e Z}, where
*±(Q..±) = ^f- + *o + O(k;1). A20)
We denote by if/r(x, t) and Ф*(х, t) the following vector-valued
functions (a column and a row, respectively): . '
ф,(х, t) = ф(х, t, QX ф:(х, t) = ф+(х, t, Q,). A21)
Using equations A05) and A12) for ф, and ф*, we find that

PERTURBATION THEORY 31
=0. A22)
Since in general E, Ф £„ for v Ф ц and the second factor in A22) is
analytic with respect to w, we find that
)^ (,23)
for any w.
Lemma 3 For any smooth function f(x) such that
f(x+ T) = wf(x), A24)
the following equalities hold:
A25)
A26)
A27)
The proof of each one of these equalities is based on considering a
contour integral of the form
с. О
where F and Ф+ represent either distinct combinations of ф„ ф? (for
A25) and the former equalities in A26) and A27)), or Е~1фь ф%, or
ЕФг> Ф\ (for the latter equalities in A26) and A27)). The contour C, is
the union of the circles of radius« centred at.P±. As follows from A16),
the residues of the integrand coincide with the terms of the correspond-
corresponding series. On the other hand, considering the behaviour of the inte-
integrand in neighbourhoods of P±, we find that S, converges to the sums
of ordinary series of the Fourier type. This proves all the equalities
A25M127).
Theorem 4 Let /,(*) and/2(x) be a pair of smooth functions that

32 I.M. KRICHEVER
satisfy condition A24). Then the functions f^x) can be uniquely repre-
represented in the form
A29)
with
т,
Moreoever, the functions/; can be represented in the form
/i => SA»^i»«
A31)
where
K.<J&zM£h. 032)
The proofs of equalities A29) with c, and A, given by A30) and A32)
follow easily from the assertions of the lemma. The uniqueness of
representation A29) follows from the 'orthogonality' relations A22).
Let 5u(x) and 8v(x) be arbitrary smooth periodic functions.
Lemma 4 For any non-resonance point Qa that differs from any of
the zeros of dp and any of the poles of ф(х, t, Q), there is a unique
constant e(t, Qo) (depending on 0 such that the equation
(Эх - {@{x, t, £„) + ?(x, t, Е0)))ф(х, t, Qo) =
has a unique solution satisfying the conditions '
Ф(х+ T, (, Qo) = wo<£(jt, t, Qo), A34)
е*>я = О. A35)

PERTURBATION THEORY 33
Proof By virtue of Theorem 4 and the normalization condition
A35), the solution ф sought for can be represented in the form
*(*,/.&>•= 2 <?,*,. A36)
Substituting A36) into A33), we find that
I (l37)
- (ё- ibuE^e-^}. A38)
By Theorem 4, the equalities define c, uniquely for v Ф 0. ё is uniquely
determined by the fact that the sums on the left-hand sides do not
contain the term with v = 0. For MOwe have
T{E-E°> A39)
(ФГоФ10Ф^2а) (ф^^0аф^^)К A40)
The lemma is proved. The result enables one to prove that a basis can
be formed from the solutions given below for the adjoint equation
ГЭ+м = 25,
[d.v = 2(cos м„)й
corresponding to the linearized Sine-Gordon equation A08). As
already mentioned a few times, to prove this assertion one only needs
to consider the conditions-ensuring that ф(х, t, Q) has no singularities
at the 'resonance points' E* and that Ф has no 'superfluous' singulari-
singularities at the poles of ф(х, t, Qo) or the zeros of dp. Let us start with the
resonance points.
For any Qefwe have w(Q) = ф~1(о{0)). Therefore, resonance
points are those points of the spectrum of the periodic and the anti-
periodic problem for the equation
A42)

34 I.M. KRICHEVER
that are distinct from any of the end points Ex of the zones. Each of the
points £, is a simple point of the spectrum, and each of the remaining
points £"„ is doubly degenerated. We shall denote by E* the
corresponding points of Г lying on different sheets of Г over Ea. The
index a varies over the set of all but n chosen pairs (N > 0, ±), which
consist of a positive integer and a sign. From the definition of w(Q) it
follows that
[^ {^y\ a = (N, ±), A43)
i.e., there are two sequences of resonance points, which tend to infinity
and to zero, respectively.
For each resonance pair of points we introduce the vector-valued
functions Ф*(х, t) whose coordinates are given by the following
formulae:
фа.г
^Ux, t,Ea)-EJ,Hx, /,£*)*,(*, /,£•*)*■'
A44)
, j г--
/ ФХх^.Е^—ф^х, t,E?)-EJt(x,(,Е:)ф2(х, 1,Е?)е-ы )
We denote by Ф(х, t, Q) the vector-valued function whose com-
components are given by the same formulae A44), but with all the
arguments E* and E* replaced by Q. In analogy with what has already
been explained in part 1 and will be explained in a more general context
in Section 2.5, there are only a finite number of linearly independent
functions among the functions in question. We can choose
ФПх, t) = Ф(х, t, E*), i= 1 л A45)
as the linearly independent functions. Finally, we define the functions
Ф{~(х, t) by the formulae '
фг(х+ T, t) = ФГ(Х, t), 0 < x < T
С = «Ж(*. U уШх, U 7i) - ФГ(х, t, ъ)ф,(х, t, 7i)],

PERTURBATION THEORY 35
tiX, t, 7,)fcCr, t, Tfc-'m ~ Ф2+(Х, t. Ъ)ф{{х, t, уУ],
A46)
«i = j J M(x> '. Tihf V.(*. r, 7i) -
Here the points y{ - yfj) e Гаге defined as the zeros of ^,@, t, Q),
MO, t, 7i@) = 0.
Theorem 5 The vector-valued functions Ф* (x, t) and #f (л-, t) form
a minimal basis in the space of square-integrable vector-valued
functions on a circle.
Proof Let &u(x) and 60(x) be arbitrary smooth functions that
satisfy the conditions
>х = 0, A47)
5>, = 0. A48)
We claim that 6u = 6v = 0.
Consider the function ф(х, t, QJ defined by formulae A36)-A39).
The function is regular everywhere except at the poles 7, of the Bloch
function ф, the zeros p£ of the differential dp, and the resonance
points. Conditions A47) ensure that ф has no singularities at the
resonance points E*. Conditions A47) with the ( + ) sign are sufficient
for ф to have at most simple poles at the points pk* or, equivalently, for
the vector-valued function
Ф(х, t, Q) = ф{х, t, Q) - ^o'f'g)^' '• Q) A49)
to be regular at the points /7k*.
Finally, the last group of conditions is equivalent to the requirement
that ф(х, t, Q) be regular at the point 7,@, which is a zero of
^i@, t> Q)- Indeed, the sum of the series
*i@, t, 7,@) = £ сЛОФЛО, 0 A50)
can be found in exactly the same way as that of G0). Conditions A48)

36 I.M. KRICHEVER
with the (-) sign imply that <£,(<), t, y,(t)) = 0.
Therefore, if Su and Sv satisfy A47) and A48), then ф(х, t, Q) = 0.
To complete the proof we find, as in part 2, that since ф = 0, it follows
that Su = 8v = 0.
The basis Ф*, Ф* is minimal since it is possible to construct the 'dual
basis' w* = (й*, м*), < = (й,*, Й,*).
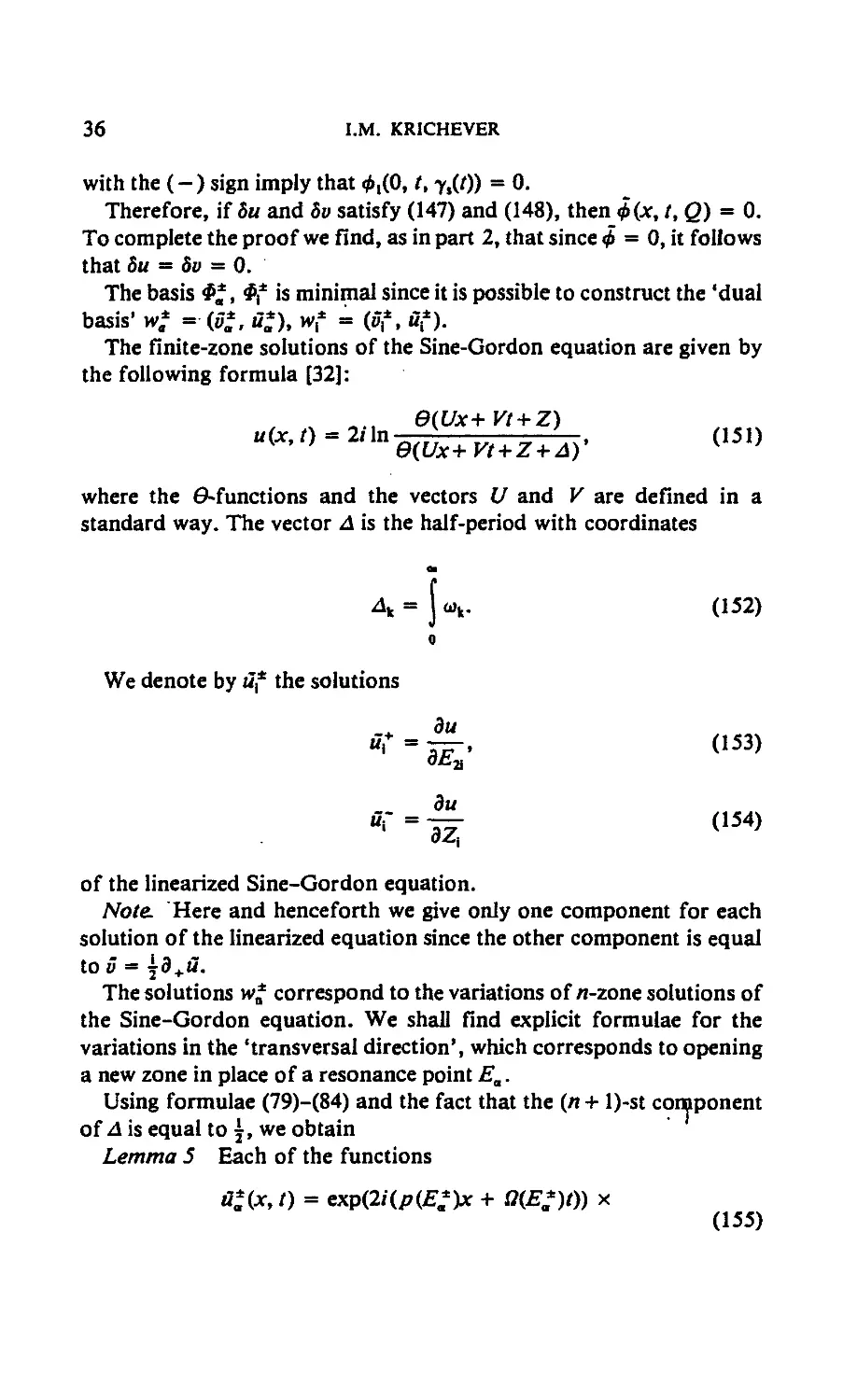
The finite-zone solutions of the Sine-Gordon equation are given by
the following formula [32]:
(,51)
where the в-functions and the vectors U and V are defined in a
standard way. The vector A is the half-period with coordinates
к- A52)
О
We denote by й* the solutions
"* =W' A53)
du
Щ = — A54)
dZ,
of the linearized Sine-Gordon equation.
Note. Here and henceforth we give only one component for each
solution of the linearized equation since the other component is equal
to v = jd+u.
The solutions w* correspond to the variations of л-zone solutions of
the Sine-Gordon equation. We shall find explicit formulae for the
variations in the 'transversal direction', which corresponds to opening
a new zone in place of a resonance point Ea.
Using formulae G9)-(84) and the fact that the {n + l)-st component
of A is equal to j, we obtain
Lemma 5 Each of the functions
utix, t) = expBi(p(E?)x + О(Е?Ю) х

PERTURBATION THEORY 37
[e(Ux+Vt + Z±2A(E*))
[ 9(Ux+Vt + Z) H
is a solution of the linearized Sine-Gordon equation. As before, p(Q)
and 17@ denote here the integrals of the differentials dp and dfl,
where
dl? = %^^— d£. A56)
dO is normalized by the same conditions as dp.
To simplify the subsequent formulations, we introduce a generalized
index a that includes (a, ±) and (/, ±), which enables us to combine
the sequences of functions w* and w* in a single symbol wu. The same
applies to Фй.
Lemma 6 The orthogonality conditions
^j^.j>, = *u&aJ A57)
hold. The constants /„ tend to zero as а -* oo.
The proof of the lemma, similarly as the proof of the analogous
assertion in the previous part of this section, is based on the fact that
given a variation of a finite-zone solution, we have
_ 4>Qc,t,Q)
о-ЖГо) A58)
for the variations of the corresponding Baker-Akhiezer functions,
where Ф is given by A36), A39), and A49) with 6v and 6u being the cor-
corresponding variations of the finite-zone solution. Comparing the
singularities on the left-hand side and on the right-hand side of
A58), we get the assertion of Lemma 6. This completes the proof of
Theorem 4.
At the same time we-obtain the following theorem.
Theorem 6 Each of the vector-valued functions w*(x, t) given by
A55) and each of the vector-valued functions w?(x, t) is a solution of
Equation A41). For each t the functions form a basis in the space of
square-integrable functions on a circle with values in the space of two-
component vectors.

2. SPECTRAL THEORY OF NON-STATIONARY
SCHRODINGER OPERATORS
2.1. The Finite-zone Case
A solution ф(х, у, wu wj of the non-stationary Schr6dinger equation
(ady - dl + и(х,у))ф = 0 A59)
with a periodic potential^ (дг,,у) = u(x+llty) = u(x,y + /j) is called a
Bloch solution if it is an eigenfunction of the operators of translation
by the periods with respect to x andy, i.e., if
, wlt w2);
,wl,w2).
It will always be assumed that the Bloch functions are normalized in
such a way that ф@, 0, wlt wj = 1. The set of pairs Q = (w,, wj such
there exist the corresponding Bloch solutions will be denoted by Г and
will be called the 'Floquet spectral set'. (For conciseness, the cor-
corresponding Bloch functions will be denoted by ф(x, y,Q),Qe Г.) The
multivalued functions p(Q) and E(Q) defined on Г by the equalities
w, = eipil, wt = еИ1 A61)
are called the quasimomentum and the quasienergy, respectively. If/"is
a smooth analytic manifold, then the differentials dp and dflare single-
valued and holomorphic. The periods of the differentials with respect
to any cycle on Гаге divisible by 2т//, and 2эг//2, respectively.
We assume that to each point Q = (w,, wj e Г there corresponds a
Bloch solution Ф*(х,у, Q) of the equation
(- аду - д? + и(х,у))ф+ = О A62)
adjoint to Equation A59) such that
2, Q) = ЩхГ(х,у, Q).
Then the following assertion holds.
Lemma 7 The equation
A64)

PERTURBATION THEORY 39
holds. (Here and henceforth we denote by <•>, and <->y the mean
values with respect to x and y, respectively.)
Equation A64) for the case of finite-zone operators A59) was
obtained for the first time in [27]. The generalization of A64) to the
case of operators of arbitrary order with matrix coefficients was given
in [14].
Proof Let ф = ф{х, у, Q) and ф* = ф+(х, у, Q), where Q and Q
are arbitrary points on Г. It follows from A59) and A62) that
Averaging this equation with respect to x and y, and letting Q tend to
Q, we get A64) with the aid of A60) and A63).
Definition Any periodic function u(x,y) such that the 'Floquet
spectral set' for the corresponding equation is isomorphic with a
Riemann surface Г of finite genus is called a finite-zone potential of
Equation A59). The functions w,(Q) and WjF). which define an
imbedding of Г into C2, are holomorphic everywhere on Г except at
one distinguished 'infinitely distant' point PQ.
Formally, the definition of finite-zone potentials applies to periodic
potentials of Equation A59) only. Nevertheless, a general definition of
finite-zone potentials can be given not only for periodic but also for
quasiperiodic potentials with a finite group of periods. By a Bloch
solution of Equation A59) with such a potential u we understand any
solution such that the logarithmic derivatives ф,ф~' and фуф'1 have the
same group of periods as u(x,y). The set of such solutions is what we
call the Floquet spectral set. If the set is a Riemann surface Г of finite
genus g < oo, the corresponding potential is called a finite-zone
potential. The fact that the definition is not void follows from the
solution of the inverse problem of reconstruction of и from the cor-
corresponding algebra-geometric data. A short presentation of the
problem, which was posed and solved in [1] and [2], is given below.
Let Г be a non-singular algebraic curve of genus g with a distin-
distinguished point Po and with a local parameter k'\Q) such that
k~ '(/>„) = 0 chosen in a neighbourhood of the point. For any sequence
of points у,, . . ., yf in general position there is a unique function
Ф(х, у, Q) such that (i) the function is meromorphic everywhere except
at Po and it has at most simple poles at the points y, (if all the points
are distinct), and (ii) in a neighbourhood of Po the function has the
form

40 I.M. KRICHEVER
Ф(х,у, Q) = eikx-"*(l + 2 Ux,y)/c~s), к = k[Q). A65)
~s), к =
Note that ф depends only on the equivalence class [к~1]г of the local
parameter. (For any positive integer m we say that k[l and k~l are
m-equivalent local parameters if the equality kt(Q) = k(Q) +
0(k~m(Q)) holds. The equivalence class of k~l will be denoted by
[k~l]m. In what follows, except for special cases where it is necessary to
make the distinction, any local parameter will be identified with its
equivalence class.)
We fix a basis of cycles щ and bx on Г with the canonical matrix of
intersections: a,°aj = b^ty = 0 and a^fy = 5^. In the standard way
(see [2] or [3]) one can define a basis of normalized holomorphic dif-
differentials <*>k> к =1,. . .,#, the vectors^ = B?,k)ofthe&-periodsofthe
differentials, and the corresponding Riemann's theta function, which
is an entire function of g complex variables and undergoes the
following transformations
9(т+ек) = 0(т); в(т+Як) = е-ив»-2"*е(г) A66)
under translations of its arguments by the unit vectors ek, which form a
basis in C*, and by the vectors Bx. Let q be an arbitrary point of Г. The
function that transforms any point QeF into the vector with
coordinates AY{Q) = \®щ is called the Abel map. For any sequence of
g points in general position 7,, . . ., 74 the function O(A(Q) + Z),
where
A67)
is the vector formed by Riemann's constants), has exactly g zeros
which coincide with the points 7, (note that by virtue of A66), the zeros
of this multivalued function on Г are correctly defined).
Let fl*", s=l, 2 denote meromorphic differentials on Г that have
single poles of the form
ia~ldk2(l
at the point Po and are normalized by the condition
fl1*» = 0. A68)

PERTURBATION THEORY 41
The vectors of the d-periods of these differentials are denoted by
2*Uk = <£ tf", 2эгКк = <E &2\ A69)
The function of the Baker-Akhiezer type ф{х,у, Q), which is defined
by its analytic properties, has the form
Vy + Z)'
The proof of A70) is just a straightforward verification of the fact that
the right-hand side remains unchanged as one passes around any cycle
on Г (i.e., it defines correctly the function ф on Г) and it has all the
necessary analytic properties.
Theorem 7 ([2]) The function ф(х,у, Q) satisfies Equation A59)
with the potential u(x,y)
u(x,y) = 23* In 9(A(P0) + Ux+ Vy + Z) - 2c, A71)
where the constant с is determined by the expansion
k(Q) + c0 + ck-\Q) + O(k-\Q)). A72)
Proof We consider the function
j , Q); u(x,y) = 2il-u(x,y), A73)
where £, is the coefficient appearing in A65). The function has all but
one of the analytic properties of ф. The expansion of the factor in front
of the exponent for ф in a neighbourhood of Po starts with a term of
order k~i rather than one, which is the case for ф. Since ф is unique, it
follows that Ф = 0. То obtain formula A71), it suffices to expand the
right-hand side of A70) in a neighbourhood of Po using the following
relation (which is a consequence of Riemann's bilinear relations):
A(Q) = A(P0) + iUk~\Q) + O{k'\Q)). A74)

42 I.M. KRICHEVER
For a curve in general position the corresponding potentials u(x,y)
are quasi periodic. The conditions defining the curves to which there
correspond periodic potentials can be formulated in the following
way.
Let dp and d£ be meromorphic differentials on Г, each having a
unique singularity at Po of the form
dp = dk(l+O(k-2)); <Ш = ia-'dk\l + О(*'3)), A75)
respectively, and each being normalized in such a way that its periods
with respect to any cycle are real. If for any cycle С on Г
£; J ,!£»£A76)
where nc, mc are integral numbers, then the periods with respect to x
and у of the potentials и corresponding to such curves Гаге equal to /,
and /2, respectively. The Baker-Akhiezer functions coincide with the
Bloch solutions of Equation A59). dp and d£ are the differentials of
quasimomentum and quasienergy, and the corresponding 'multipliers'
wx(Q) and w2(Q) are equal to
= exp
Q Q
[ //, ] dp j; w2(Q) = exp (//, { d£ J. A77)
\ я I \ q I
(Conditions A76) ensure that w(Q) are independent of the path of
integration.) The proof of the assertions stated follows from the fact
that
.О), (П8)
Их, У + 1г, Q) = w2(Q№(x, У, Q), A79)
since the right-hand and left-hand sides of these equalities have the
same analytic properties.
The formally adjoint or dual Baker-Akhiezer functions, which
satisfy Equation A62), can be defined in the following way. \
Let dfi be the unique meromorphic differential on Г with a single
second-order pole at Po and with zeros at y,, . . ., yt. Apart from the
zeros at the points 7,, dQ has g other zeros, which will be denoted by
■V+ -V*

PERTURBATION THEORY 43
Ф*(х, у, Q) is called the dual Baker-Akhiezer function if it is mero-
morphic on Гexcept at Po and has poles at 7* y*. In a neighbour-
neighbourhood of Po the function has the form
Ф+(х,у,О) = е-***''**1 I + 2jK(x,y)k-'\. A80)
Lemma 8 ([56]) For the coefficients £, and £,+ jn expansions A65)
and A80), the equation
Ш.У) + K(x,y) = 0 A81)
holds.
Proof It follows from A65) and A81), and from the definition of
Ф* that the differential
dD(x, y, Q) = ф(х,у, QW+(x,у, Q)dH(Q) A82)
is ho'lomorphic everywhere except at Po, where it has a second-order
pole. Thus, the residue of d/?at Po is equal to zero. Since the residue is
equal to the left-hand side of A81), the lemma is proved.
Corollary The dual Baker-Akhiezer function ф* is a solution of
Equation A62), which is formally adjoint to Equation A59), the latter
being satisfied by ф.
Lemma 9 If Г, Po, and 7,, . . ., yt are such that the corresponding
potential и is non-singular, then
° A83)
Proof Let us note that it suffices to prove the assertion for periodic
potentials since the set of such potentials is dense (as I, -* 00) among all
finite-zone potentials.
We consider an arbitrary periodic variation bu of u. In analogy with
the proof of A64) (see also [14] and [27]) one can find that
A84)
It follows that the zeros of < фф* >„ and <фхф* — фф* )у cannot coincide.
Otherwise <<^бн^+» = 0 at the point where both of these functions
equal zero (where «•>> is the mean value with respect to x, y), which
cannot be the case for an arbitrary perturbation bu. It follows from

44 I.M. KRICHEVER
A64) that the right-hand sides of A83) are holomorphic everywhere
except at Po, have zeros coinciding with the poles of ф, ф*, and a
second-order pole at Po. Since these properties define do uniquely, the
lemma is proved.
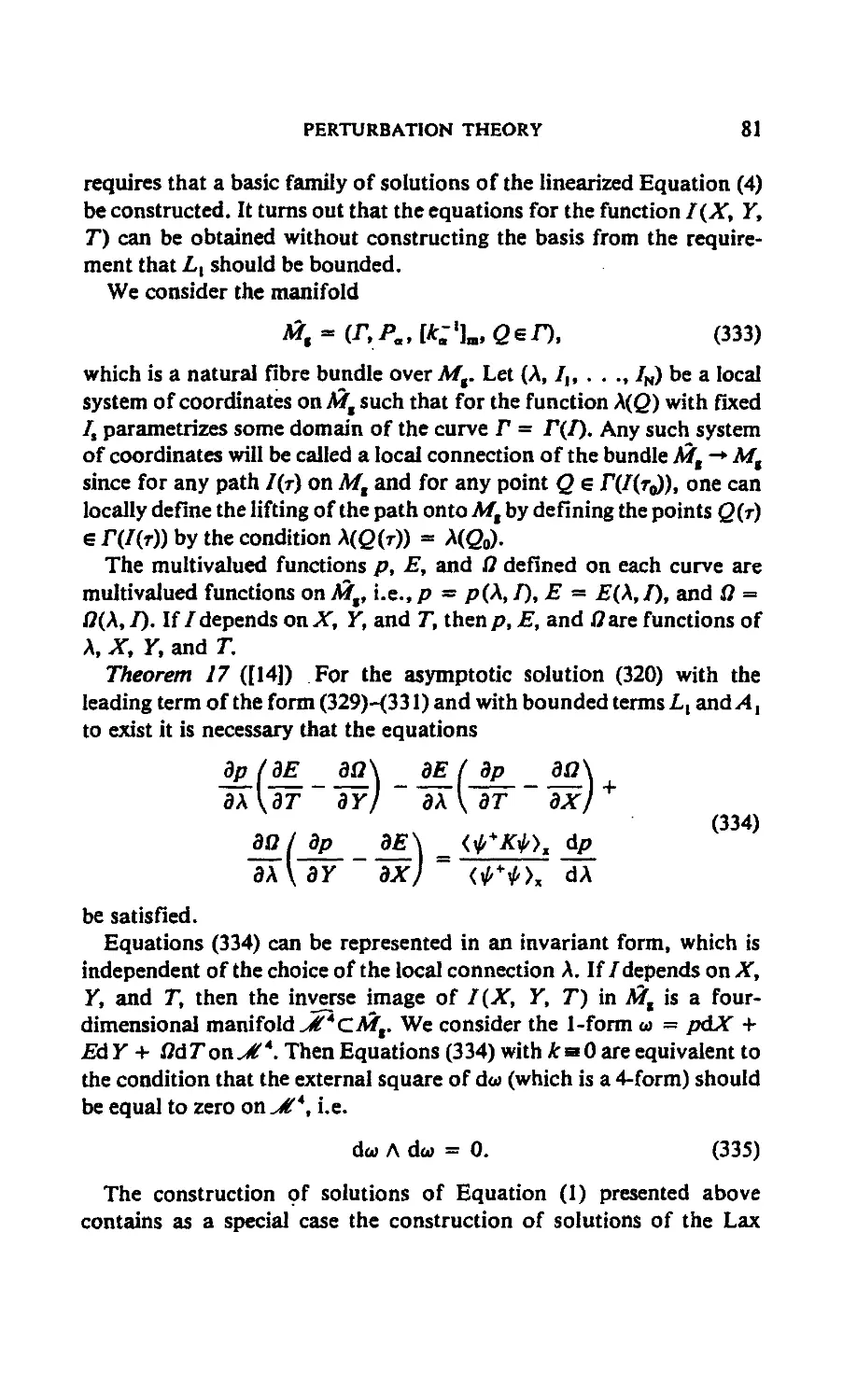
Theorem 8 For any smooth real-valued finite-zone potential и of
Equation A59), the corresponding curve Г is isomorphic with the
Floquet spectral set.
Proof Let w10 be a complex number such that the equation wx{Q^
= wl0 has simple roots only. Thus, in complete analogy with the proof
of Equations E0) and E1), we find using A81) that for any smooth
function f(x) such that /(*+/,) = wlof(x), the equality
A85)
holds with фа(х, у) = ф(х,у, &) and ф;(х,у) = ф+{х, у, QJ.
Let ф{х,у, wl0, w2) be a Bloch solution of Equation A59). Being the
mean value between two solutions of adjoint equations, each of the
expressions <.ф£ф)х is independent of y, and so it can be nonzero only if
w^ = wz(Qn) = w2. Thus, (w10, riy coincides with one of the points Qa.
The theorem is proved.
The potentials и corresponding to any of the sequences (Г, Po, *"',
7,) are complex-valued meromorphic functions. There is a funda-
fundamental difference between the conditions ensuring that the poten-
potentials are real-valued and non-singular in the cases where a=\ and
a=i.
The case a = i. For и to be real-valued it is necessary that there be an
antiholomorphic involution т on Г such that t(PJ = /V The local
parameter k~l should be chosen in such a way that k(r(Q)) = k(Q).
Under the action of r the poles y, should be transformed into the dual
sequence r(yj = 7,*, i.e., the differential dffwith a single second-order
pole at Po should have zeros at 7, and r(gs).
If these conditions are satisfied, then
ФЧх,У,0) = Ф(х,У,т<2)), \ A86)
because the analytic properties of these functions are the same. It
follows that ^(x,y) = %t(x,y) and u = 2/£u is real-valued.
For и to be smooth it is sufficient that the anti-involution be of

PERTURBATION THEORY 45
dividing type, which means that the invariant ovals a0, . . ., a,, / < g
divide Г into two domains Г*. If the differential dQ corresponding to
7 y, is non-negative on a, with respect to the orientation defined
on these ovals, which are regarded as the boundary of Г*, then и has
no singularities for real values of x and y.
The sufficiency of the above conditions for и to be smooth was first
proved in [38]. The necessity of these conditions has been proved
recently in [39] on the basis of a detailed analysis of A71) involving
theta functions. Below, we give a brief outline of another method of
proving the necessity.
First we note that it suffices to prove the necessity of the conditions
in question for periodic potentials, for the set of curves Г with a distin-
distinguished point Po that correspond to these potentials is dense among all
smooth curves with distinguished point as L, -+ oo.
For a=i the Floquet spectral set is invariant under the
transformation
(W1.W2)-(wf1, wf1). A87)
Since the set is isomorphic with Г, A87) generates an antiholomorphic
involution т: Г-* Г. The invariant ovals for т divides Г into two
domains: Г*, where | w, | > 1, and Г", where | w, | < 1. dp is positive
on these ovals and, by virtue of A83), so is dQ. The assertion is proved.
The invariant ovals a0, . . ., a, of the anti-involution r form the
'spectrum' of the operator A59) in the space of square-integrable
functions on a straight line.
Theorem 9 ([127]) Let the parameters Г, Po, k~\ and 7, satisfy the
above-mentioned conditions, which ensure that the corresponding
finite-zone potential u(x,y) is real-valued and non-singular. Then
8(x-x')
A88)
(UaJ\P0
In a more general situation the theorem was proved in [27] by the
standard method of contour integration.
Let us note that for Qea,, the functions ф{х,у, Q) and ф+(х,у, Q)
are the complex conjugates of each other and they are bounded since
|w,(Q)| =1.
The case 0= 1. Finite-zone solutions of Equation A59) with 0= 1 are

4Ь I.M. KRICHEVER
real-valued and non-singular if and only if the data (Г, Pa, k~l, 7,)
defining the solutions satisfy the following conditions: there is an anti-
holomorphic involution т on the curve Г with g+ 1 invariant ovals
(such curves are called M-curves); exactly one of the points Po, 7,,. . .,
7, lies on each of the ovals invariant with respect to r. and the local
parameter k~ X(Q) in a neighbourhood of Po is chosen in such a way that
k(r(Q))=-k(Q)._ _
Note т*йр - - dp and r*dE = - dE on the invariant ovals. There-
Therefore, the condition ensuring that the periods of these differentials are
real means that each of the integrals of dp and dLE along a,, . . ., ax
vanishes. Thus, in the case where a—\ the differentials dp and dLE
coincide with the differentials C" and 0a) defined at the beginning of
this part of Section 2.
2.2. Perturbation Theory for Formal Bloch Solutions
We begin here the study of the Bloch functions for the operator in A39)
with a general periodic potential.
The gauge transformation ф -* е"*лф, where dyot(y) is a periodic
function, transforms each of the solutions of A59) into a solution of
the same equation but with a different potential п = u(x,y) - odya. It
follows that the spectral sets corresponding to и and й are isomorphic.
Therefore, in what follows we restrict ourselves to the case of periodic
potentials that satisfy the condition
<u(x,y))x = 0. A89)
The main aim of this part of the paper is to construct the theory of
perturbations for formal Bloch solutions of Equation A59). This will
enable us to express the solutions by means of basic families of Bloch
solutions Фа(х,у) of the non-perturbed Equation A59) with some
potential uo(x,y). Let us give more details.
We fix a complex number w,. A sequence of Bloch solutions
.У) = Ф(х,У, GB), Qa = (w,, w^ e Го A90)
of Equation A59) with и = ио(х,у) will be called a basic sequence if
any continuously differentiable function/(дг) such that
wj(x) A91)
can be represented as a convergent series of the form

PERTURBATION THEORY 47
Дх)= %]тл(у)фа(х,у). A92)
л
As follows from the results of the previous part of the section, a basic
sequence can be formed from the Bloch solutions of Equation A39)
with any finite-zone potential щ. The simple case where u0 s 0 serves
as an important example. In this case the functions
фв = exp(/*Bx - a ' lkly), A93)
where кя are the roots of the equation
w, m elkJ\ i.e. ka = k0 + ^ n A94)
м
form a basic sequence for any complex number щ.
Apart from фв, we need the 'dual sequence'
t: = t+(x,y,QJ
of Bloch solutions of the formally adjoint equation
(<т6у+Эх2 - uo(x, у))Ф: = 0 A95)
such that the orthogonality conditions
are satisfied.
Being able to use the sequences фа and ф*, one can easily construct a
Bloch solution ф(х,у,О) of Equation A59) in the 'non-resonance
case', i.e. in the case where the condition
ww * w2o, n Ф 0 A97)
is satisfied. The solution can be constructed in the form of a formal
series
Ф (x, y, Qo) = £ Ux, у, Qo), ф0 = ф0. A98)
The series describes the 'perturbation' of the Bloch solution ф0 of the
non-perturbed equation. (Here and henceforth series of the type of

48 I.M. KRICHEVER
A98) are understood as expansions in powers of a formally small
parameter 5U.)
Lemma 10 If condition A75) holds, then there is a unique formal
series
&) A99)
j* i
such that the equation
(ady - Э* + Щ + 5м) Пх, У, Go) - Р(У, Qo) Пх, У, Qo) B00)
has a formal solution of the form
OB
, y, Qo) - 2 Ф,(х, У, Qo), Фо = Фо = Ф(х, У, Qo), B01)
1-0
which satisfies the conditions
(W?>, = (^W,. B02)
/„ у, Qo) = w, Пх, у, Qo);
, >» + /2, Qo) = w20lf(j:, >», Qo).
The corresponding solution is unique. The terms of the series B01) and
the quantities Fs are given by the recurrent formulae B05)-B09).
Note that since B03) holds and Fis unique, it follows that F(y, Qo) is
a periodic function with respect to y.
Proof Equation B00) is equivalent to the system of equations
(ady - dl + ио)Ф, = 2 F>*.-i ~ bu*>-.• B04)
i-1
Since ф„ form a basic sequence, the functions Ф, required can be repre-
represented in the form
Ф, - 2 сХУ> Qo)Ux,y), c°a = 6„,0. B05)
л
Condition B02) is equivalent to the fact that '
Co' = 0,s>l. B06)
Substituting B05) into B04) and comparing the coefficients cor-

PERTURBATION THEORY 49
responding to фп for n Ф 0 in the expansions in terms of фа of the right-
hand side and the left-hand side of the equality, we find that
B07)
The equation combined with the condition wln сЩу + /j) = tv^e^).
which is equivalent to B03), defines cn5 uniquely (and so it also defines
It follows from B06) that the coefficient corresponding to ф0 in the
expansion of the right-hand side of B04) is equal to zero. Thus,
B09)
The proof of the lemma is completed.
Corollary The formula
<-!■
\ 9
и, &> = «р [ - 1 F(y\ Q0)dy • -jgftff BЮ)
defines a formal Bloch solution of Equation A59) such that
',&>), B11)
г» У + d, Qo) = *ъФ ix, у, Qo), B12)
where the corresponding multiplier w^ is equal to
In the stationary case, where и is independent of y, the foregoing
formulae are replaced by the standard formulae of the theory of
perturbations of eigenfunctions corresponding to simple eigenvalues.
As already mentioned above, condition A97) is an analogue of the

l.M. KK1CHEVER
condition ensuring that an eigenvalue of an operator is simple. In cases
where the condition is violated, it is necessary to follow a procedure,
which is analogous to that in the theory of perturbations of multiple
eigenvalues.
As the set of indices corresponding to resonances, one can take any
finite sequence of integral numbers /e Z such that
w*. * Wb.ael.nel. B14)
(Till the end of this part of Section 2, integral indices belonging to /will
be denoted by Greek letters! while all other indices will be denoted by
Latin letters.)
Lemma 11 There are unique formal series
my. *.)
such that the equations
{ad,- дгх +uo + bu)W(x, y, w,) = ]TJ F${y, wt)*в(х, У, wt)
в
B16)
have formal Bloch solutions of the form
*."t*.У, wi). Фо" = Ф(х,У, Qa), B17)
s-0
%x + l»y. w,) = w, *"(x,y, wt), B18)
+ 12, wt) m w^V^y, w,), B19)
satisfying the conditions
= 5a,e<^+^e>x. B20)
The corresponding solutions ♦" are unique and are given by
B21M223).
The proof of the lemma is completely analogous to that of Lemma 7,
which is a special case of Lemma 11. Therefore, we give only the final
formulae for /•£ and for the coefficients of the series
<№.V. >*i) = 2 <-a{y, wMx,y),s>l. B21)

PERTURBATION THEORY 51
We have
. B23,
We define the matrix TJfty, wt) by the equation
oTy + TF = 0, 7*@) = 1. B24)
A formal solution of this equation can be found in the form
OB
T(y, Щ) - S T,(y, w,), To = 1, B25)
1-0
where Ts,s^ 1 is given by the recurrent formulae
о
Each of the functions
wx)FUy. Щ)йУ. B26)
B27)
is a solution of Equation A59). Under translation by the period with
respect to*, the functions are multiplied by wu and under translation
by the period with respect to у they are transformed in the following
way:
$""(*. У + /2, ",) = £ 7?(w.) ¥(x, y, w,); f = Г(/2 w,). B28)
/>
It is natural to call the finite family of formal solutions $"* the 'quasi-
Bloch family', since it is invariant under translations by the periods
with respect to x and y. The characteristic equation
JWv) = 0 B29)

52 I.M. KRICHEVER
is an analogue of the 'secular equation' in the standard theory of
perturbations of multiple eigenvalues.
Corollary Let ha(wlt wj be an eigenvector of the matrix fg (w,)*^
normalized is such a way that
E A.«2)**@, 0, w.) = 1; Q = (w,, tfj. B30)
О
Then
Ux,y, Q) = 2 *«(£>*-(*, .У, *.) B31)
is a formal Bloch solution of Equation A59) with multipliers w, and w2,
where w2 is a root of Equation B29). Moreover, B31) is normalized in
the standard way.
By analogy with the foregoing procedure, one can construct formal
Bloch solutions for Equation A62), which is formally adjoint to
A59).
Lemma 12 If conditions B14) are satisfied, then there are unique
formal series
such that the equations
have formal Bloch solutions of the form
Ф?"(х,у, w,); фда = ф+{х,у, Qa),
satisfying the conditions 1
The corresponding solutions are unique and are given by formulae

PERTURBATION THEORY 53
which are completely analogous to B21)-B23).
We define the matrix Tg"(y, w,) by means of the equation
-ОТ? + T+F+ = 0, 7-@, *>,) = 1. B32)
Then each of the functions
&+'(x,y, w,) = 2 Т$а(У, wt)*+8(x,y, w.) B33)
в
is a solution of A62). Under translation by the period with respect to x,
the functions are multiplied by w,, and under translation by the
period with respect to у they undergo the following transformations
**'&.y, ",), B34)
в
?Ч»0 = t+(/г, щ).
Corollary The equality
2 f;t;e m ь** B35)
у
holds.
Since if and %r*B satisfy equations which are formally adjoint to
each other, the quantities < &*"&">, are independent of y. Since 7"@)
= T*@) = 1, it follows that
B36)
Thus,
Corollary The formal Bloch solutions of Equation A62) are
defined on the surface given by Equation B37) and have the multipliers
wf' and w2~'.
2.3. The Structure of the Riemann Surface for Bloch Functions
We shall consider here the formal perturbation series constructed
above with wo = O chosen as the non-perturbed potential. The Bloch

54 I.M. KRICHEVER
solutions of the 'unperturbed' equation A39) and the adjoint Equation
(ad, - dfWx, у, к) = О, (аду + д?)фЧх, y,k) = 0 B38)
can be parametrized by the points of the complex Аг-plane and have the
form
The corresponding eigenvalues of the operators of translation by /, and
/г with respect to x and у are equal to
w, = eMl, wz = e-~>k4' B40)
respectively. As has already been mentioned above, for any complex
number k0, the functions фп = Ф(х,у, ка), where
*„ = *o + ^ B41)
form a basic sequence for continuously differentiable functions f(x)
that satisfy condition A91) with wl0 = wt(k0). The dual sequence ф£ =
Ф*(х, у, kj satisfies condition A95),
<*Ж;>, = 5B>m. B42)
It follows that B01), B05), B08), B09), and B10) with 6u replaced by
u(x,y) define formal Bloch solutions of Equation A59) if k0 satisfies
condition A97) ensuring that there are no resonances, which we shall
now consider more carefully.
It follows from B40) that for щ = 0 there are only simple
resonances, that is, the equations
B43)
may have no more than two roots ArA) and ki2). The corresponding pairs
of resonance points have the form
*0) = *n,m. *m - *-n.-m. B44)
where
kNM = -j- + —— -—-, N Ф 0, M being integral numbers. )B45)
Thus, if

PERTURBATION THEORY 55
*o = *n.m B46)
does not hold for any integers N Ф 0, M, then there is a formal Bloch
solution of Equation A59).
Looking ahead, we remark that using significantly simpler estimates
than those obtained in [9], one can show that for sufficiently small
potentials u(x,y) that admit analytic continuation to some neigh-
neighbourhood of the real numbers x, y, the perturbation series converge
outside some neighbourhood of the resonance points B45), and in this
domain of convergence they define a function Ф(х,у,к0), which is
analytic with respect to kQ. This is true for any value of a. The basic
difference between the cases Rea = 0 and Rea Ф 0 manifests itself even
for small u(x,y) if one tries to perform the analytic continuation of ф
into the 'resonance domain'. The fact that it is impossible to perform
such a continuation (at least by the methods developed in the present
article) for Rea = 0 is connected with that in this case the points kNM are
everywhere dense on the real axis. It would be very interesting and
important to find a language which would enable one to describe the
situation in a neighbourhood of this continuous resonance set. We
shall return briefly to this question again.
If Rea Ф 0, the resonance points kNM have only one limiting point
к = oo. This fact is the key to all subsequent constructions. To the end
of the present part of this section we restrict ourselves to the case where
a=l, although all the assertions (in particular Theorem 4) proved for
complex-valued potentials u(x,y), are valid for any Rea Ф 0. For
a = 1, the case of real-valued periodic potentials и (х, у) is distinguished
in a natural way. In this case the general assertions admit further
significant effectivization.
The perturbation series for formal Bloch functions are constructed
under the assumption that u(x,y) is formally small. It turns out that
the series converge in various domains for an arbitrary periodic func-
function u(x,y) that admits analytic continuation into some neighbour-
neighbourhood of the real numbers x, y. The proofs of the following assertions
require direct, but technically cumbersome estimates of the terms of
the corresponding series. The estimates can be found in [9]. In the
present survey we restrict ourselves only to the formulation of the basic
assertions and some comments.
We assume that u(x,y) admits analytic continuation into the domain
|Im;t| <т„ |Im^| <r2, in which it is bounded by a constant U, i.e.,

56 l.M. KR1CHEVER
\u{x,y)\ < U, \lmx\ ^ r,, \Imy\ < тг. B47)
Lemma 13 There are constants No and « depending on С/, т„ and тг
only such that in the domain R being the complement of the neighbour-
neighbourhoods /?NM of the resonance points
jRe(k - *NM) | < £, | Im(* - *NM) | < 1 B48)
and the central resonance domain
\Rek\ HN0, \lmk\ ^ No B49)
the perturbation series constructed by virtue of Lemma 10 and its
corollary are absolutely and uniformly convergent in R and define the
Bloch solutions ф{х,у, kg) of Equation A59) (<j= 1), which are non-
vanishing and analytic in the domain k9ей, |1тдг| ^ rlt \lmy\ < r2.
The 'large' central resonance domain Ro appears in the formulation
of the lemma as a result of the fact that u(x, y) is not small, and so all
the eigenvalues w2a in the central domain are 'indistinguishable modulo
I/,' despite the fact that there are only double resonance points.
The problem of the continuation of Bloch functions into the interior
of resonance domains can be divided into two stages. First, quasi-
Bloch functions are constructed.
Let k0 &R0, but let it belong to one of the neighbourhoods RNM. The
function w{(k) defines a mapping of both #NM and /?.N,.M onto the
same domain of the complex plane of the w, variable. We denote
the image by /?fN|,|M|. As the resonance set / in B14) we choose {0,
-IN}. Then the series given in Lemma 11 define the functions
V(x,y, и»,) and F%(x,y, и»,), which are analytic with respect to и», 6
/f|N,,M| and satisfy A96). The matrix T(yt tv,) defined by B24) is also
analytic in /?|Ni>|M|. It follows that each of the Bloch solutions of
Equation A58) defined by formulae B30) and B31) for each point of
the two-sheet covering J?)N)>|M, over i^|Nia{M| given by the equation
w*2 - WiSpifgiwJWjg) + det^w,)*^) = 0, B50)
Wj = w^o), koeRNM, a, 0 « 0, -IN
is a meromorphic function on ^|N|,|Mf
The poles of ф{х,у, Q) coincide with the poles h", and so they are
independent of x, y. The constants N9 and e in Lemma 13 can be chosen
in such a way that the discriminant of Equation B50) can vanish only

PERTURBATION THEORY 57
inside the domain Л|М|,|м|- This assertion follows from the fact that the
assumptions of Lemma 13 as well as the necessary conditions for У
and Ff to be analytic are satisfied on the boundary of RNM and /?_Ni_M.
From the construction of the Bloch solutions ф(х,у, k0) and ф(x, y, k$
with iv, = wt(k0) = tv,(&Q) it follows that the passage to these solutions
corresponds to the process of diagonalization of the matrix ff(w^)wv.
Therefore, the eigenvalues of the matrix coincide on the boundary with
the values йг(к0) and #2(ОД defined by B13) for the non-resonance
domain. The old eigenvalues w2(k0) and w2(ko) were distinct on the
boundary of the domain. Simple estimates show that the magnitude of
perturbations of the eigenvalues is less than the difference between
them. It follows that Equation B50) has distinct roots on the boundary
of Л|М||М| and its discriminant can have zeros only inside the domain.
All the assertions proved above hold for any potential that satisfies
conditions B47). In particular, the assertions hold for potentials of the
form uT = ти(х, y), where 0 ^ т ^ 1. Since the number of zeros of the
discriminant inside the domain is preserved under such a deformation,
and for т = 0 the discriminant has one double zero at jvJ*m = w,(A:NM),
we conclude that the discriminant of Equation B49) has either two
simple zeros or one double zero.
Definition A pair of integral numbers (N > 0, M) such that kf^ e
R will be called a distinguished pair if the discriminant of Equation
B50) has a double zero.
In this case ^|N|,|m| is reducible, i.e. it breaks up into two sheets.
Moreover, the Bloch function ф(х,у, к0) admits analytic continuation
into the domains RNM and /?_N_M, which are the separate sheets of
^|n|.|m|- For any pair that is not distinguished, /?|ni.|m| is a non-singular
two-sheet surface.
Lemma 14 The Bloch function ф*(х,у, Q) has one simple pole on
■^|n|.|m| (f°r апУ Ра*г N > 0,M that is not distinguished).
From the topological point of view, 'pasting in' the two-sheet
covering surface /?)NfJM| in place of the two domains RNM and R _Ni_M,
so that the Bloch function ф can be analytically continued from the
non-resonance domain to ^|N|,|M|> represents simple 'surgery', which
consists in 'attaching a handle' between two resonance points kNM and
*-N,-M-
We consider the continuation of ф~ to the interior of the central
resonance domain R9 given by inequalities B49), such that one can
assume without loss of generality that N£ = /,/2т No is an integer. The

58 I.M. KRICHEVER
function и», given by A91) represents Ro as an No' -sheet covering of the
annulusexpf-Ay,) < w, < ехр(ЛГ0/,) in the tv,-plane.
As the family of resonance indices / for tv,, which satisfy the
foregoing inequalities, we choose all indices such that \Reka\ < No,
Exactly as before, we find that ^(x, у, кй) can be analytically continued
from the non-resonance domain to the Riemann surface Ro defined
over the annulus exp(-7V0/,) < iv, < exp(Ngl,) by the characteristic
Equation B29) with a matrix of dimensions BjV0' x 2jV0'), namely, the
monodromy matrix of quasi-Bloch solutions constructed as perturba-
perturbations of the solutions exp(ikax-kly) of the free Equation B38). This
matrix, which is denoted by tgiw^Wjg, is analytic with respect to w,
in its domain of definition. In this way we arrive at the following
lemma.
Lemma 15 The Bloch function ф(х,у,кй) admits analytic con-
continuation from the non-resonance domain to ft0, where it is a mero-
meromorphic function whose poles are independent of x, y. The number of
poles g0 does not exceed the number of pairs (N > 0, M) such that
kNM e Ro. In a general situation where /?0 is non-singular, g0 is equal to
the genus of Ro.
Looking ahead, we point out that for real-valued potentials u(x,y),
the surface Ro is always non-singular.
We denote by Г the Riemann surface obtained from the complex
A-plane by 'pasting-in' Ro instead of Ro, and /?|N|.|m| instead of /?NM
and J?_n,_m (for those pairs N > 0, M that are not distinguished). The
surface is smooth everywhere, except perhaps at a finite number of
points in Йо.
Notation So far the Bloch solutions of Equation A59) constructed
with the aid of perturbation theory have been denoted by \f. In what
follows we shall omit the tilde so that the solutions will be written as
Ф(х,У, Q)- Analogously, we shall omit the tilde over the eigenvalues
w2(Q) of the operator of translation by the period with respect to y.
Theorem 10 The Riemann surface Г is isomorphic with the
'Floquet spectral set' for the operator in A59). The Bloch solutions
w(x, y, Q) of this equation normalized by the condition tv@,0, Q) = 1
are meromorphic on Г. The poles of ф are independent of x, y\ ф has
one simple pole in each of the domains /?|N|.|M|> and it has g0 poles in
.£„, where g0 is equal to the genus of /?0 in the general solution of non-
singular Ro. Outside ^|N|.imi anc* Д> the function ф is holomorphic and
has no zeros.

PERTURBATION THEORY 59
All the assertions of the theorem, except the first one, follow from
the construction of Г. To each point Q e Г there correspond the eigen-
eigenvalues w{(Q) and w2(Q) of the operators of translation by the periods
with respect to x and y. They define a mapping from Г into C2 with
coordinates w, and w2.
The fact that an isomorphism between Г and the Floquet spectral set
is thereby established can be proved in complete analogy with the proof
given in part 1 of this section for the finite-zone case.
Once more, let us stress the fact that the theorem holds for all
potentials (including complex-valued ones) that satisfy condition
B01). For real-valued potentials u(x,y) the theorem can be stated in a
more effective form. Before doing this, let us give the following
definition.
Definition u(x,y) is called a finite-zone potential if all but a finite
number of pairs (N > 0, M) are distinguished for this potential, i.e. Г
is a curve of finite genus.
For finite-zone potentials the corresponding surface Г outside a
bounded domain coincides with a neighbourhood of infinity on the
ordinary complex plane. Therefore, it admits compactification by a
single 'infinitely distant' point Po= ». In what follows we shall retain
the symbol Г to denote the algebraic curve corresponding to the
compact Riemann surface.
Corollary TheBloch solutions ф(х,у, Q), QeFoiEquation A59)
with a finite-zone potential и are defined on the compact Riemann
surface Г. The function $ is meromorphic everywhere except at the
distinguished point Po and has g poles, which are independent of x, y,
where g is equal to the genus of Г in the general situation of non-
singular Г. In a neighourhood of Po, Ф(.х,у, Q) has the form
ф = e^-^f 1 + S SM,y)k-') B51)
with A: = k~ \Q) being a local parameter in the neighbourhood of Po.
All the assertions of the Corollary, except the last, follow directly
from the definition of finite-zone potentials and from Theorem 10. To
obtain B51), we use the fact that in a neighbourhood of infinity, ф can
be represented by means of the perturbation series for the non-
resonance case. It follows from the estimates of the perturbation series
that the function

60
I.M. KRICHEVER
»+*, B52)
which is holomorphic in a pierced neighbourhood of Po> 's bounded.
Thus, the function is holomorphic in this neighbourhood and can be
expanded into the following series:
B53)
1-0
It follows from the normalization condition A89) that £„= 1, and so
the corollary is proved. The assertion of the corollary means that the
class of periodic finite-zone potentials given in the first part of the
section is identical to that in the present part.
A family*- = {(a'.A")} ofpairs of complex numbers, wheresvaries
over a finite or an infinite subset of pairs of integral numbers {N > 0,
M), will be called admissible if
B54)
and the intervals [p>, p?\, which are parallel to the imaginary axis, do
not intersect each other.
For each admissible family ir we construct a Riemann surface Дт)
by making vertical cuts between the pairs of points/7,', p3" and -p't,
-Pt, and pasting together the left-hand edge of the right-hand cut and
the right-hand edge of the left-hand cut, and vice versa:
As a result, to each pair of cuts (p,\ p,") and (-/?,', -^
corresponds a cycle, which is not homologous to zero. The cycle will be
denoted by a,.
Theorem 11 For any real-valued periodic potential u(x,y) that
admits analytic continuation to a neighbourhood of the real numbers x

PERTURBATION THEORY 61
and y, the Bloch solutions of Equation A59) with a=\ can be
parameterized by the points Q of the Riemann surface Г{ж) for some
admissible family ж. The corresponding function ф{х,у, Q) is mero-
morphic on Г(*г) and has one simple pole on each of the cycles a,.
Proof For real-valued potentials u, complex conjugation trans-
transforms each Bloch solution of Equation A59) into a Bloch solution of
the same equation. Thus, the mapping
r: (w,, Wj) - (iv,, wj B55)
is an antiholomorphic involution of the Floquet spectral set, which, by
virtue of Theorem 4, induces an antiholomorphic involution of the
'spectral curve' Г. One can also see directly from the construction of Г
that such an anti-involution exists. In particular, from the formulae of
Lemma 10 it follows that т is of the form k0-* -k~0 and ф(х, у, *,) =
Ф(.х,у, - JO in the non-resonance domain.
We consider the neighbourhoods RN M of the resonance points lying
outside the central domain Ro. The fact that /?|N|,|mi is invariant with
respect to r means that either both of the zeros of the discriminant of
Equation B49) lie on the straight line Re к = xN/llt or they are
arranged symmetrically outside the line. The latter is impossible,
because the imaginary parts of the eigenvectors of the operator of
translation by the period with respect to у have different signs on the
intersection of the straight line with the boundary of RNM (for the free
Equation B38) this can be seen directly from B40)). It follows that
inside the interval of the straight line y/Re к — tcN/Ix there is a point
where w2 is real. Therefore, both of the zeros of the discriminant,
which we denote by p't and p,", lie on the straight line Re к = тЛ///,.
The cut between the zeros corresponds to the cycle a, introduced by the
requirement that both tv, and wx be real on the cycle. This cycle is a
'forbidden zone' which appears in place of the resonance point k,. We
claim that the pole of the Bloch function lies on а,, ф and ф+ are real-
valued on this cycle. Since ф(х,у, Q) = Ф(х,у, r(Q)), the poles of ф
must be invariant with respect to r. Because there is only one pole of ф
on /?|N(,|m|. tne pole must be invariant with respect to r, which means
that it belongs to the invariant cycle a,.
The theorem is proved for sufficiently small potentials u(x,y) such
that the central domain Ro is empty. We shall increase u(x,y). The
structure of Г described above is topologically stable and can be
destroyed only if the cycles a, with different indices s merge with each

62 I.M. KRICHEVER
other (in which case Г has a singularity). The periodicity condition for
и acts as an obstacle that prevents the cycles from merging with each
other. The requirement that и be periodic with respect to x keeps apart
any cycles a, and a't such that N Ф N'. What prevents the cycles over
intervals of the same straight line Re к - irN/ll from merging with
each other is the periodicity of и with respect to y. If one cuts Г along
the cycles and along the straight line Re к = 0, then a single-valued
branch of quasienergy E(Q) is defined in the domain Re к > 0. Since
the differential d£is purely imaginary on a,, the real part of E(Q) can
be extended by continuity to a,, where it is identically equal to itM/l2,
where s = (N, M). Therefore, the cycles a, are separated by the values
of the real parts of quasimomentum and quasienergy and cannot merge
with each other. Therefore, the theorem being proved holds for all u,
not only for small ones.
From the construction of Г it follows that for sufficiently large
\s\ = \N\ + \M\, the pointsp't andp',' belong to the neighbourhoods
R, of the resonance points kt, as stated in B54). In the case in question
with the potentials и being analytic in some neighbourhood of the real
numbers x, y, one can prove that
|A'-A"I = O(e-"|N|-"M|). B56)
The representation of Г described in Theorem 11 is well known (see
[40, 41]) in the spectral theory of the Sturm-Liouville operator with a
periodic potential u(x). The corresponding curves Гаге hyperelliptic.
There are the sequences p't and p," with s = (N, 0) associated with the
curves, such that p's = pi'. As independent parameters, which
determine и uniquely, one can choose a, = hap', and the points 7, so
that one of the points belongs to each of the cycles. In terms of these
parameters, the process of approximation of и by finite-zone potentials
u0 appears to be very simple. Each potential ua corresponds to a family
of data such that df = d%, \s\ < G, df = 0, and \s\ > G ([53]).
Such an approach to the proof that an arbitrary periodic potential
can be approximated by finite-zone potentials is much more difficult in
the non-stationary case since the parameters p'% and p'i are not
independent (they were dependent also in the stationary case, but the
relations between them were explicit). As shown earlier, to any finite
admissible family there correspond finite-zone potentials which are
periodic with respect to x and quasiperiodic with respect to у (see part 1
of this section). As a result of the periodicity condition with respect to

PERTURBATION THEORY 63
у, only half of the quantities p't and p" are independent (for example,
p't or p', -p't). Therefore, to construct a process of approximation by
periodic finite-zone potentials, one has to 'slam' the zones [pl,p','] for
large \s\, adjusting simultaneously the other zones. In principle, this
way is possible, but it is technically rather difficult to realize. Below we
give a proof of the theorem on approximation based on another idea,
which is also applicable in the case of the spectral theory of operators
such that the poles of the Bloch functions do not lie on the invariant
ovals of the corresponding anti-involution (this includes the spectral
theory of the operators used to construct finite-zone solutions of the
Sine-Gordon equation or the non-linear SchrOdinger equation with
repulsion, etc.). Since the parametrization of и by admissible families т
will not be explicitly used in the proof, we do not specify more precisely
the necessary and sufficient conditions for a family to be admissible.
2.4. The Theorem'on Approximation
Let the potential щ(х, у) of Equation A59) with Re а Ф 0 be a trigono-
trigonometric polynomial. Since
, . ,., , BviNx 2viMy\
, У, Wtf+(*. У. *-n.-m) = exp _—+—_£ B57)
\ 'I '2 /
(in this part of this section we adhere to the original definitions and
notation used in part 1 and at the beginning of part 2, i.e. ф(х,у, к)
denotes a solution of the free Equation B38), and ф(х, у, Q) denotes a
solution of an equation of the type A59)), it follows that for some G,
«ФОс.У.кн*М>Чх.У,к_ъ-и>и1Р,У)» = 0, \N\ + \M\ > G.
B58)
From Lemma 10 it follows that under condition B58), the first-order
perturbation term Ф{(х,у, kj does not have poles at the resonance
points £NM for \N\ + \M\ > G and can be extended by continuity to
these points. The poles at these points appear already in the next order
of perturbation theory. The basic idea of the construction below is
based on the fact that it is possible to construct a formal series U(x,y)
with the leading term u, and with the subsequent terms chosen in such a
way that the corresponding terms of the perturbation series do not have
poles at AKiM for \N\ + \M\ > G.

64 I.M. KRICHEVER
Lemma 16 Let u,(x,y) be a periodic function that satisfies
conditions B58). Then there is a unique formal series
OB
U{x,y) = E".(^^) B59)
s-l
such that for s^2, the equalities \N\ + \M\ < G and
и™ = «ф(х,у,ккм)ф+(х,у,кш)и,(х,у))> - 0 B60)
hold, and for any kg Ф- ANM, where \N\ + |A/| < G, there is a unique
formal series
ее
ЯЛ*о)=Е^-*о) B61)
such that the equation
{ad, - dl + U(x, у)) Пх, У, К) - F(y, *„) Пх, у, к0) B62)
has a formal solution of the form
, y, *o) = 2 <М*> J'. *o). ^o = ^ (Jf. >". ^o). B63)
which satisfies the conditions
<Пх,У, W+(x,y,k^x m 1, • B64)
h, У, k0) =
y.j;
, у + 1г, Аго) = и-(*) ^(л: j/ *) ( }
Proof * Equation B62) is equivalent to the system
s
(аду-Э;)ф, = S № - «i)*,-i. B66)
For Ло * kNM the terms of the series B61) and B63) are given by
the following formulae, which are completely analogous to B05)-
B09): \
o) = S <^o+ uA-i>,, К « ^ + (*, J', *o), B67)
i-l

PERTURBATION THEORY 65
,У), Ф„ = Ф(х,У, k0 + -^-), B68)
я#0
B69)
We assume that the terms щ of the series B59) with << 5-1 are
constructed in such a way that <t>S.x,y, k9) have no poles at ko = kt№i for
\N\ + \M\ > G. This means that also at these points the functions ф1
can be defined by continuity. The next term u,(x,y) of the series B59)
can be found from the conditions
'f*
NM
= E (
~ <*+(x,У, *.к.
B70)
Equations B70) combined with the normalization conditions B60) and
A89) define all the coefficients of the Fourier series for the periodic
function ut(x, y). It follows from B70) that ф,(х,у, кд) has no poles at
it^for \N\ + \M\ > G. The lemma is proved.
Theorem 12 Any smooth periodic potential u(x,y) of Equation
A59) with Rea Ф 0 that admits analytic continuation into a neighbour-
neighbourhood of the real variables x, у can be uniformly approximated along
with any number of derivatives by finite-zone potentials.
For any integral number G we denote by u°(x,y) and u°{x,y) the
periodic functions such that
u(x,y) = u°{x,y) + u°(x,y); <ио°>х - <«1°>x = 0, B71)
and such that B60) holds for wo° and B58) holds for и,0. By virtue of
Lemma 16, to u° there corresponds the unique formal series V°(x, y)
given by B59).
The proof of the theorem given in [9] can be reduced to the proof
that there is a constant Go, which depends on the quantities U, r,, and r2
appearing in B47), such that for G > Go the corresponding formal
series B59) converges and defines the finite-zone potential Ц°(х,у) of
Equation A59). It turns out that if |1тдг| < r, and |Imj/| < r2) then
\u{x,y)-U°{x,y)\

66 I.M. KRICHEVER
where the constant Mis independent of U, т„ and т2. It follows that the
sequence of finite-zone potentials U°(x,y) converges uniformly to
u(x,y) along with any number of derivatives as G -* «.
2.5. The Theorem on Completeness for Products ofBloch
Functions
In this part of Section 2 we restrict ourselves to the case of real-valued
non-singular finite-zone potentials of Equation AS8) with <r=l. As
shown above, the potentials are determined by an M-curve Г with a
distinguished point Po e a0 (where a0, . . ., at are the invariant ovals of
the anti-involution т) and a set of points 7,ее,. Moreover, the
potentials depend on the equivalence class [k~l]2 of a local parameter
such that k(r(Q)) » - k(Q). The real dimension of the manifold
Mt = (r,P0,[k-lW B72)
of such data is equal to 3g+l, where g is the genus of Г. The
submanifold M\ consisting of those elements of B72) that correspond
to potentials with vanishing mean values with respect to x is of
dimension 3g. As can be seen from A71) and A72), and the fact that
dp = fl*" (for a= 1), the submanifold can be defined by the condition
P~\Q) 6 №~ 'bt wherep(Q) is an arbitrary branch of quasimomentum.
The main aim of this part of section 2 is to construct a Fourier basis
in the space of periodic functions with respect to x and у from products
of Bloch functions that correspond to periodic finite-zone operators,
and of the dual analogues of such Bloch functions. Before presenting
the results, we need some detailed information about the structure of
the 'resonance points' on the curves that correspond to the potentials in
question.
Let the data (Г, Po, [k'1]^) e Ml satisfy conditions A75), which are
necessary and sufficient for и to be periodic. Then the functions w{(Q),
/=1,2, which represent the eigenvalues of the operators of translation
by the periods with respect to x and y, are defined on Г. A pair of points
Q and Q' is called a resonance pair if w^Q) = w-,(Q').
On each of the domains Г* obtained by dividing Г by the cycles
a0 at one can choose a single-valued branch of the integrals')
Q Q
p(Q) = j dp, E(Q) = J d£, qea0. B73)

PERTURBATION THEORY 67
(As Г we chose the domain where Rep > 0.)
Lemma 17 ([10]) For any M-curve Г the mapping
Г+ э Q - (Rep(Q), ReE(Q)) B74)
is a real diffeomorphism of Г* onto the right-hand half-plane R1 with
g points being pricked out. Г and Po correspond to a periodic potential
of Equation A59) with <r= 1 if and only if the coordinates of the points
are of the form (тА/,/,"', тМ,/2"'), where N,>0 and M, are integral
numbers. All the pairs of resonance points on Г are of the form Р£м,
where РЦМ = т(Р^м), and P^M is the inverse image of the point with
coordinates (iMf1, rMl{1), where N and Mare integral numbers such
that (N, M) * (N,, M.) for 5=1, .... ^ and N>0, under
transformation B74).
Let ф(х,у, Q) be the Baker-Akhiezer function constructed from
B72) and from a set of poles 7,. If the corresponding potential satisfies
the periodicity condition A76), then each of the products
*nm(*. У) = Их,У, P&tW* (x, у, Л?м) B75)
is a periodic function of x and у by virtue of the definition of resonance
points. Each of the products Ф(х,у,О)ф+(х,у, Q) is also a periodic
function. It follows from the Riemann-Roch theorem that among the
latter products there are only g+1 linearly independent ones. Indeed,
for any x and у, фф+ is meromorphic as a function of Q and may have
poles at the points у„ yt+ only. By the Riemann-Roch theorem, the
dimension of the space of such functions is equal to g+ 1. (It follows
from this discussion that the dimension of the space of the functions
Ф(х,у, Q№*(x,y, Q) does not exceed £+1. In the proof of Theorem
13 it will be shown that the dimension is equal to g+ 1.)
We denote by Ф*(х, у) the periodic functions
Ф?(х,у) = Их,У, РЖ(х,У, Ръ), B76)
where P}, j= 1, . . ., 2g are the zeros of the differential dp, which are
numbered in such a way that P^., and Ръ lie on a,.
Let L\ = 1%(Т*) be the space of square-integrable functions that are
periodic with respect to x and у and have vanishing mean values with
respect to x. We denote by (i.^)* the dual space. We shall define
elements Ф~ е (L%)*, which, as shown later, can be combined with Ф£м
and Ф* to form an analogue of the Fourier basis in
We define т,(дг, у) by the formula

68 I.M. KRICHEVER
^ B77)
which coincides up to the constant factor G(A(P0) + Z) with the
coefficient corresponding to the singular term in the expansion of
t(x,y,Q) with respect to the local parameter 9{A{Q) + Z) in a
neighbourhood of its pole. (We recall that G(A(yJ + Z) = 0.) Let Q'
denote the points of Г such that Щу^ = w,(Q^). We consider the
series
, у w,(Qn') Ф,у)Г{х,у,<2$
"гЫ -sCQD <Ф(х,У, Q'BW+(x,y QD>'
Lemma 18 For all x and .у < 1г, the series B78) converges and
defines a smooth analytic function Ф~(х,у), which is periodic with
respect to x. For any continuously differentiable function v(x,y) with
periods /, and /2 with respect to x andy, there exists the limit
B79)
which is finite and defines the element #t~ e (££)*.
Proof As | л | -» oe, we have k{Q$ = p, + 2тл//,. Thus,
Analogously,
Л.ч / Irinx 4ггпгу 4тлр,^\ „ois
Ф:<х-.у. Q'J ~ exp — + —j-i + —рЧ B81)
up to a finite factor. If follows that the terms of the series B78)
decrease exponentially for у < l2. Фг is periodic with respect to x since,
by virtue of the definition of Q*t all the terms of the series are periodic
with respect to x. We denote by т,° the periodic function t
t,° = t,(x, y)cxp( - ip,x- iEsy),
where p, and E, are the values of quasimomentum and quasienergy at
y,. We have

PERTURBATION THEORY 69
B82)
Thus, the left-hand side of B79) can be represented as the sum of a
series whose terms with \n\ > No can be uniformly estimated by means
of the Fourier coefficients of the periodic function T°(x,y)v(x,y),
which yields the last assertion of the lemma.
Theorem 13 The functions Ф? and Ф^м form a minimal basis in
(L\)*.
The proof of the theorem is completely analogous to that of
Theorem 1 (Section 1). First, we prove that for any continuously dif-
ferentiable periodic function v(x,y), it follows from the equalities
h
(а) «»Ф,+ » = 0; (b) J <Ф;0с,у)я0с,у)>£у = 0,
B83)
(a) «»#5m» = 0; (b) <»>, = 0 B84)
that ioO. (Here and henceforth «•» denotes the mean values with
respect to x and y.)
For any Qoersuch that w,(£H) Ф wt(P), Qo Ф ys, and Qo * P£M,
we consider the series
„Ф0
<285)
where ф„ = ф(х,у, QJ, w2a = w2(Qa), and, as before, Qa is defined by
the condition w,Bn) = w,(Q0). The series B85) converges and defines
an analytic function of Qo. It follows from B84) that the function
admits analytic continuation to all resonance points PNM. We claim
that it can be extended by continuity to the points Qo Ф Pt such that
Wi(Qo) = w,(Pj). Consider ф{х,у, Qo), where Qo is close to Qo. Letting
Qo tend to Qo, we find that among the terms of expansion B85) there
are two terms which tend to infinity. The terms correspond to the
indices /i0 and i^ + 1 such that the corresponding points Q^ and QBt+, lie
in a neighbourhood of Pr (The terms tend to infinity since their
denominators <^„,^£> and <^n,+ ,^+,> tend to zero as Qo -» Q0.)The

70 1.М. KRICHEVER
sum of these two terms tends to a finite limit. Indeed, for n Ф 0 the
terms of B85) are identical with the residues at the points QB of the
differential
f . , f
J У J
, Q)i4x',y', Q)Ux\y\ QMx\?)dx
B86)
which is locally a smooth function of Go- Thus, the sum of the two
terms of B85) that tend ttTinfinity converges to the integral of the
differential B85) along a small contour encompassing Pr
Therefore, if a satisfies condition B84(a)), then Ф(х,у, Qo) is an
analytic function on Г except at the points Pv y,, and the distinguished
point Po. The function may have simple poles at the points y,, and it
may have double poles at the points P)t j=\ 2g. From the
equality
r+h r r
Wu \ X.(y'W=—^^— [xniy'W- \Xady\
B87)
it follows that the function
Ф{х,У, Go) = ф{х,у, Go) - «МО, 0, <2Жх,У, Qo) B88)
has no poles at the points Pt if v satisfies conditions B83(a)). It follows
from B83(b)) that ф@,0, Go) has no poles at the points y,. This means
that ф is meromorphic on Г except at Po and may have simple poles at
the points 7,. It follows from B85) that
,y, Go№+С*.У, Go) = 0(*-'(Go)). B89)
Therefore, ф is a function of the Baker-Akhiezer type, but the factor in
front of the exponent in the expansion of ф starts with a term of order
O(k~l). Since the Baker-Akhiezer function is unique, we conclude that
ф = 0.
As shown earlier, \рп = ф(х, у, Qn) is a basic sequence (in the sense of
the definition in part 2 of this section). Comparing formulae B05) and
B08) with B85), we find that

PERTURBATION THEORY 71
(Эу - дгх+щ)ф (х, у. Go) = -»*.+ тттут5 *•» <290>
where щ is a finite-zone potential corresponding to the Baker-
Akhiezer function ф(х,у, Q). Since ф = 0, the left-hand side of B94) is
equal to zero. Then it follows from B83(a)) that v ■ 0. The complete-
completeness of the family Фf, #йм is proved.
The proof that the family is minimal follows from the construction
of the 'dual' basis in L\ given below. We consider an arbitrary variation
u{x,y; t) of the finite-zone potential u0 = u(x,y; O). For any Q Ф P},
Phm>- we denote by Q(t) the point of the Riemann surface Д
corresponding to the potential u(x,y;r) that is defined by the
condition wt(Q(T)) = w,(£H). We set
фг = фт(х, у, Go) = дтф{x, у, Q{t)) I T-o. B91)
By definition, the function exhibits Bloch's behaviour with respect to x
with the multiplier w,(Q0).
Lemma 19 For any variation u(x,y, т), the function ф(х,у, Q)
given by B85) with
o(x, у) = дти{х,У, t)Ir_e B92)
is equal to
The right-hand side of B97) is a Bloch function with
multipliers w,0 and wx and satisfies the normalization condition
(ФФоУ* = 0- Differentiating A59) with respect to r, we find that the
function is a solution of Equation B90). As shown in part 2 of this
section, the solution is unique and is given by B85). The lemma is
proved.
First, we consider finite-zone variations that preserve the periods of
щ. Among such variations there are those that do not change Г and do
not move the poles 7, of the Bloch function. We set
i>;(x,y) = — u(x,y\Г, 7,, . . ., 7l). B94)
(These functions are linear combinations of ди/dz,, where и is given by
A71) and Z-, are the coordinates of the vector z.) Besides, there are

72 I.M. KRICHEVER
variations that preserve ys, but not Г. For example, if the end-pointspu
of the cuts in the model of Г in part 3 of this section are chosen as the
parameters defining Г (we recall that in the case of variations of Г
preserving the periods of u0, only half of the end-points of the cuts are
independent), then the functions
K(x,y) = — и(х,у\рг,. . .,pu, 7,, . . ., 7,) B95)
°Р
can be defined.
Lemma 20 The functions vf satisfy the following equations
«»,**Йм» - «tf*№*» = 0, B96)
«»,+ 4V» = 0, «»;#,t» = 0, B97)
«0,4*» = 6„., «»,-*,"» = asSe.rа, Ф 0. B98)
Proof For both of the types of variations considered, фт (where r
stands for 7, от p^ has no poles at the points /*nm- Hence, equalities
B96) follow. The function d$/dyt has a double pole at the point 7,, and
it has simple poles at 7,. for s * s'.At the remaining points the function
is analytic. Comparing these properties with those that follow from
B85), we obtain the latter pair of equalities B97) and B98). For the
variations of pu, each of the derivatives дф/др^ has a pole atpj,. Hence
we obtain the former pair of equalities B97) and B98). The lemma is
proved.
According to the assertion of the lemma, the functions Ф* form a
basis in the tangent bundle of the manifold of periodic finite-zone
potentials corresponding to curves of genus g. We shall show below
that the functions #^u are dual to the variations that are transversal to
the manifold, under which 'a zone is opened' in place of the resonance
points P&,.
We consider small neighbourhoods R^M for any pair of points Р^м-
Each of these neighbourhoods can be identified my means of the
function w, with some neighbourhood R^ of the point w,(.PnM). If the
notation P±(wl) e R&f, wl(P±)=wl is used for w, e R^,, then the
functions w^(w,) = w^P*) are analytic in Лмм. Let /?NM be the two-
sheet covering of Лцм defined by the equation
Z2 - (w2+(n',)+ w2-(w,))z + A -e2)w;(Wl)w;(Wl) = 0. B99)
For sufficiently small e, the boundary of /?NM breaks up into two

PERTURBATION THEORY 73
circles, and each of the circles can be naturally identified with the
boundary of R^m- This identification makes it possible to paste
together the domain /?цм and the complement of the domains /?Йм т Г.
As a result, a Riemann surface of genus g+1 is obtained. We denote
the surface by Ц,м. The involution r can be naturally extended onto
Г£м, where it has a new invariant cycle at+l e Г^м in addition to the
old invariant cycles a0,. . ., at. Using formulae G9-83) to evaluate the
variation of the finite-zone potential A70), we find that for the
functions
with
the assertions of the following lemma hold.
Lemma 21 The functions »Йм satisfy the following relations:
= 0, «0ЙмФ,*» - О, C01)
0,
Proof Considering the derivative of the Bloch function with
respect to e, we find that the corresponding function ф( has simple poles
at the points y, and Pt, and poles at the pair of points Pnm- Comparing
the residue at these poles with B85), we obtain C02). Equalities C01)
follow from the fact that \p, has simple poles at y, and Pu.
The lemmas proved enable one to draw the conclusion that Ф* and
#nm form a minimal basis in (Lf)*. At the same time, the following
theorem is proved.
Theorem 14 The functions vf and v^ defined by B94), B95), and
C00) form a minimal basis in L\.
3. PERIODIC PROBLEM FOR KADOMTSEV-PETVIASHVILI
TYPE EQUATIONS
3.1. Perturbation Theory for Finite-Zone Solutions of KP-2
The construction of finite-zone solutions of the Kadomtsev-
Petviashivili (KP) equation [1, 2] differs from the construction of

72 I.M. KRICHEVER
variations that preserve 7,, but not Г. For example, if the end-points ръ
of the cuts in the model of Tin part 3 of this section are chosen as the
parameters defining Г (we recall that in the case of variations of Г
preserving the periods of u0, only half of the end-points of the cuts are
independent), then the functions
vtix.y) = — и(х,у\рг pu, 7,, . . ., 7,) B95)
°Р
can be defined.
Lemma 20 The functions of satisfy the following equations
<<»*#nm)) = <@»*^nm)) ~ 0» B96)
« К #,:» = о,«0; ф; » = о, B97)
«»,+ Ф,*>> = 5И., «»,"#,:» = atba., a, * 0. B98)
Proof For both of the types of variations considered, фт (where r
stands for 7, ог/?^) has no poles at the points Р^м- Hence, equalities
B96) follow. The function дф/ду, has a double pole at the point 7,, and
it has simple poles at 7,. for 5 Ф s'. At the remaining points the function
is analytic. Comparing these properties with those that follow from
B85), we obtain the latter pair of equalities B97) and B98). For the
variations ofp^, each of the derivatives дф/Ъръ has a pole at/^. Hence
we obtain the former pair of equalities B97) and B98). The lemma is
proved.
According to the assertion of the lemma, the functions Фf form a
basis in the tangent bundle of the manifold of periodic finite-zone
potentials corresponding to curves of genus g. We shall show below
that the functions Ф£м are dual to the variations that are transversal to
the manifold, under which 'a zone is opened' in place of the resonance
points Pnm-
We consider small neighbourhoods R£M for any pair of points PJiM-
Each of these neighbourhoods can be identified my means of the
function w, with some neighbourhood Rtm of the point №,(P,JM). If the
notation P*(»v,) e Л^м» щ{Р*)—Щ is used for wt e i?NM> then the
functions wf(w{) = w2(P*) are analytic in i?NM. Let /?цм be the two-
sheet covering of RHM defined by the equation
Z1 — (H'1f(w,) + iv2"(w,))z + A —е2)и'2+(и'|)и'2"(и'1) = О. B99)
For sufficiently small e, the boundary of RNM breaks up into two

PERTURBATION THEORY 73
circles, and each of the circles can be naturally identified with the
boundary of R^M. This identification makes it possible to paste
together the domain Лмм and the complement of the domains R£M in Г.
As a result, a Riemann surface of genus g + 1 is obtained. We denote
the surface by Ц,м. The involution r can be naturally extended onto
Г^м, where it has a new invariant cycle a1+, 6 J^,M in addition to the
old invariant cycles a0,. . ., at. Using formulae G9-83) to evaluate the
variation of the finite-zone potential A70), we find that for the
functions
with
NM
the assertions of the following lemma hold.
Lemma 21 The functions г>£м satisfy the following relations:
*.*» = 0, «i&,*,*» = °. C01)
» = 0, «»&€#&,,» = Wmm,- C02)
Proof Considering the derivative of the Bloch function with
respect to e, we find that the corresponding function \pt has simple poles
at the points y, and Pit and poles at the pair of points P^M. Comparing
the residue at these poles with B85), we obtain C02). Equalities C01)
follow from the fact that ф, has simple poles at 7, and Ръ.
The lemmas proved enable one to draw the conclusion that Ф* and
#nm form a minimal basis in (Z.£)*. At the same time, the following
theorem is proved.
Theorem 14 The functions vf and v^, defined by B94), B95), and
C00) form a minimal basis in L\.
3. PERIODIC PROBLEM FOR KADOMTSEV-PETVIASHVILI
TYPE EQUATIONS
3.1. Perturbation Theory for Finite-Zone Solutions of KP-2
The construction of finite-zone solutions of the Kadomtsev-
Petviashivili (KP) equation [1, 2] differs from the construction of

l.M. KRICHEVER
finite-zone potentials for the non-stationary Schrodinger operator only
by the introduction of one more variable in the argument of the
exponential function in the definition of the Baker-Akhiezer function.
The solutions of the KP equation can be constructed with the aid of the
Baker-Akhiezer function ф(х, у, t, Q), which is meromorphic on Г
except at Po, has poles at 7,, . . ., 7,, and is of the form
+ 2jUx,y,t)k-), C03)
к = k(Q)
in a neighbourhood of Po.
ф can be expressed in terms of theta functions in complete analogy
with A70):
C04)
ijw>4yo*io> 9(A(Q) + Ux+ Vy + Wt+Z)
X 6(A(P0) + Ux+ Vy+ Wt + Z) *
9(A(Q) + Z) '
where Я0* and /20' are the same as in Section 2, and QP* is a normalized
Abelian differential with a pole of the form d/:J at Po. The cor-
corresponding finite-zone solution u(x,y, t) is given by the formula
u(x,y, t) = 23?In 9(Ux+ Vy+ Wt+A(PJ) + Z) + const. C05)
We consider the problem of constructing asymptotic solutions of the
equation
i \ ± = 0, C06)
where e is a small parameter and K{u) is a differential polynomial.
There are several formulations of the problem. One of the
formulations is connected with the study of the effect of the perturbing
term on the solution of the periodic problem for the KP-2 equation. In
this case an asymptotic series is constructed for the solution of the
Cauchy problem with initial conditions u(x,y,Q) belonging to a
neighbourhood of a finite-zone solution of the KP-2 equation.
Another formulation of the problem is valid in the case where A"=0. In
this case an asymptotic solution of the KP-2 equation is sought for such
that the initial term is equal to

PERTURBATION THEORY 75
uo(x,y, t) = 2dl\n 6(rlS(X, Y, T)\I(X, Y, T)) + c(X, Y, T).
C07)
where
u{z) = 2d\In 9(z\ I), Э, = E £/,3, C08)
is a periodic function of г = (г г,) whose parameters (i.e. the
matrix of periods of hoiomorphic differentials on Г) depend on the
slow variables X=ex, Y-ey, and T=et. The vector-valued function S
is defined by the equations
3XS = U{X, Y, T), dYS = V{X, Y, T),
dTS = W(X, Y, T),
where U, V, and W are the vectors representing the periods of the dif-
differentials dp, d£, and dfi. The vectors depend on X, Y, and Г through
the basic parameters (Г, Po, k~l) regarded as functions of these
variables.
For spatially one-dimensional systems, in particular for the KdV
equation, most attention was devoted to the latter formulation of the
problem [12, 13, 42]. Combining these two problems, we shall seek a
solution of Equation C06) in the form
u{x,y,t) = uo(x,y,t\X, Y, T) + ЛеЧфс,у,ЦХ, Y, T). C10)
/-1
To construct the formal series in the case where щ is a periodic
function of xandy, it suffices to construct a family of solutions of the
linearized Equation C06)
2уу | х = 0 C11)
such that for all / the family constitutes a basis in the space of periodic
functions with respect toxandj. In addition, it is necessary to find the
dual basis consisting of solutions of the adjoint linear equation
$ ! * = 0. C12)
To construct a solution of Equation C11), we use the fact that for a
family of solutions of the non-linear equation, the derivatives of the
solutions with respect to the parameters satisfy the linearized equation.
It follows that the functions

76 I.M. KRICHEVER
д д
К(x,y,t)=-r— uo(x,y,t); v*(x,y,t) = — uo(x, y,t), C13)
ор ду,
where щ = щ(х,у, t\ylt p^ are finite-zone solutions given by A61),
satisfy Equation C11).
We obtain the following assertion by considering variations of Г
which are analogous to those used in part 5 of this section and cor-
correspond to 'attaching a handle' between Q and t(Q).
Lemma 22 Each of the functions
v(x,y,t,Q) =
0(Ux+Vy+Wt + Z)
is a solution of Equation C11). Here r{Q) is a real-valued function
defined in the following way. Let wQ be a normalized differential of the
third kind with residues ± 1/2*/ at Q and t(Q). We have
l C15)
as Q' - Q.
According to the definition of resonance points, each of the
functions «nm = «(х.д'./.Р^,) is a periodic solution of Equation C11).
We denote by Ф*(х,у, t) the functions constructed with the aid of
Ф(х,у, t, Q) and ф*(х,у, t, Q) in exactly the same way as the functions
Ф*(х,у) in the last part of Section 1. Moreover, we define the periodic
functions Ф$м = Ф(х,у, t,P£M), where
Ф(х у t Q)
In complete analogy with the results of part 5 of Section 1 we obtain the
following theorem.
Theorem 15 The functions o* and v^, form a basis in L\ for any /.
Moreover, these functions and Ф*, Ф£м satisfy the orthogonality

PERTURBATION THEORY 77
conditions B97, 298, 301 and 302). The formulae for v(x,y, t, Q) and
*(•*» У'. Л Q) obtained above enable one easily to define all the terms of
the series C10) in the case of a periodic solution u0. Direct analysis of
the resulting expressions shows that the corresponding series can be
defined for all finite-zone solutions by approximating the solutions on
any compact set by finite-zone periodic (with respect to x and y)
solutions such that the periods /,, /2 -» ». In this approximation, the set
of points Qeas for which there are integral numbers p = (r,, . . ., rg)
such that
Rep(Q) - т,£/, + .. . + rtUt,ReE(Q) - r,K, + . . . + rtVt. C17)
turns out to be the limit of the subset of resonance points giving non-
trivial contributions to щ. Let R = R(U, V) be a subgroup in Z* that
consists of sequences of integral numbers such that the right-hand sides
in C17) are equal to zero. For any sequence p e Z', we denote by ~p the
corresponding element of the quotient group Z*/R. The points
described in B00) are uniquely defined by those classes ~p (in what
follows, we denote the points by Qf) that are distinct from zero and
from any of the classes pf, where pf is a sequence such that r; = ±5U.
We denote by Е\щ, . . ., u{.t] the 'deficiency' of order e' obtained by
substituting the corresponding partial sum of the series C10) into C06).
Theoreml6 The term щ(х,у,(\Х, Г, Г) of the series A93) is equal
to
У
Щ = 2 (c^(t)v*(x,y, t) + cr(t)v;(x,y, t))
J-l
C18)
Here
C19)
Ф, Qf) = q(G>) - ] «*Ocy, t\Qf)dxi
0

Note that in C19) only the dependence of the terms on the 'fast'
variables x, y, and / is shown, although each of the terms is also a
function of the slow variables X, Y, and ^involved in the definition of
a,, v, Ф*. and Ф by means of the parameters Г, Po, and k~\ which
depend on these variables. Moreover, the constants of integration c*
and 6i(Qf) in C19) may also depend on X, Y, and T.
The most interesting moment is when one defines the dependence of
the basic parameters Г, Po, and A: for finite-zone solutions on X, Y,
and T, starting from the requirement that the leading correction term
u, must be uniformly bounded with respect to /. The next part of this
section is devoted to this problem.
3.2. Whitham Equations for Spatially Two-dimensional
'Integrable Systems'
The problem of constructing asymptotic solutions of general spatially
two-dimensional equations A) and their perturbations can be posed in
the following way. Let K(A) be a differential operator of order m - 1
whose coefficients are differential polynomials of the coefficients of an
operator A. We seek asymptotic solutions
A = Ao + eA{ +. . „L = La + eL, +.. . C20)
of the equation
dtL - dyA + [L,A]~ eK(A) = 0. C21)
The construction [2] of finite-zone solutions of Equation C03) starts
from a non-singular algebraic curve Г of genus g with distinguished
points Pa, a— 1, . . ., / and with local parameters k~'(<2) such that
К l(Qa) — 0 given in some neighbourhoods of these points. We set Ra
— E/L| Л<ак1 (where Лы are the constants used along with the constants
г£, which are the diagonal elements of the leading coefficient of A, to
parametrize the systems of equations of the KP type).
For any sequence of points 7,, . . ., 74+)_i in general position there
is a unique function Фа(х,у, t, Q), QeF such that (i) the function
is meromorphic on Г outside the set of points Pa and has poles at
the points y, (at most simple poles if the points 7, are distinct); and
(ii) in a neighbourhood of Pg the function can be represented in the
form

PERTURBATION THEORY 79
where £0°* = 5^ and *e=
We denote by ф (x, y, t, Q) the vector with coordinates фа. As proved
in [2], there are unique operators L and A of the form B) with
(Ix /)-matrix coefficients such that
(dy-L)Hx,y, t, Q) = 0, (dt-A)>Kx,y, t, Q) = 0. C23)
Since equalities C22) hold for all Q, the operators L and A satisfy A)
(with a= 1). Since ^e are unique, it follows easily that they do not
change under transformations of local parameters such that k't - ks +
O(kg"). Local parameters connected with each other in this way
belong to the same equivalence class [£/']„,.
The complex dimension of the manifold consisting of the sequences
Mt = (Г, Pa, [k; ']„), where Г is of genus g, C24)
is equal to N = 3g - 3 + (m + 2)l. One can introduce a complex-
analytic structure on Mt. Let / = (/„ . . ., /N) be an arbitrary (local)
system of coordinates on Mt. All the quantities in the subsequent
formulae are complex-analytic functions of /.
We denote by dp, d£, and dfi the meromorphic differentials on Г
with poles of the form dka, dRa(ka), and ifadk^ at the points Pa,
respectively, uniquely normalized by the requirement that their
integrals along any cycle be real. Let a,, b} be a canonical basis of cycles
on Г. We define the real vector U with coordinates
p, *= 1 g. C25)
By analogy with &E and dfi, we define the 2^-dimensional real vectors
V and W. By cutting Г along the cycles ax and b}, one can choose a
single-valued branch of the integrals p(Q), E[Q), and Q(Q). In a
neighbourhood of Pa the branches are of the form
p m ka - aa + O{k;\ E = Rm{ke) -b %
a = cc - ca + O(k:1)
p, E, and О can be uniquely normalized by the condition c,
0.

80 I.M. KRICHEVER
It was proved in {14] with the aid of explicit formulae involving theta
functions that the constructed finite-zone solutions have the following
form: if a = a(I), b = b(I), and с — c(I) denote the diagonal matrices
aJaff, bj^, and c.,6^, then
C27)
where g = exp(i(ax+by + ct+<f>)), «^ = #„5^, and the coefficients tf|
and $i of the operators £ and A are of the form
tf, = щ(их+ Vy + Wt + Z\I), oj - Oj(£/x+ Vy + Wt+Z\I). C28)
Hereflj(zi,. . ..z^J^and Wj(z zu\I) are functions whose periods
with respect to the variables z, are equal to one. The real coordinates of
Z and the complex constants Фа can be determined from the sequence
Yi. • • -, T.+i-i-
In order not to encumber the presentation with unnecessary
technical details, we refer to [14] for the details of the construction of
the explicit formulae for й, and vr In accordance with the general
ideology of the Whitham method (the non-linear WKB method), we
shall consider asymptotic solutions C19) such that the leading term has
the form
Ao = GA0G-\ Lo = GL0G'\ C29)
where G - exp(«e-'S0(A', Y, T) + i<&(X, Y, Г)), and the coefficients
of the operators Ao and Lo are equal to
SjirlS(X, Y, T) + Z(X, Y, ТЦЦХ, Y, T)), C30)
, Y,T) + Z(X, Y, T)\I(X, Y, T)). C31)
The vector-valued function S and the diagonal matrix So should satisfy
the conditions
a,s = U(X, y, T), dYs = v(X, y, T), dTs = w{x, y, t),
*x50 = a(X, Y, T), dyS0 = b(X, Y, T), drS0 = c{X, Y,
where U, V, and Ware the vectors representing the periods of the dif-
differentials dp, dE, and dfl defined on the curve fthat corresponds to the
data C24), which are parametrized by I(X, Y, 7"). The diagonal
matrices a, b, and с are defined in C26).
As for the example of the KP-2 equation analysed above, the
complete solution of the problem of constructing the entire series C20)

PERTURBATION THEORY 81
requires that a basic family of solutions of the linearized Equation D)
be constructed. It turns out that the equations for the function I(X, Y,
T) can be obtained without constructing the basis from the require-
requirement that Lx should be bounded.
We consider the manifold
Mt = (Г,Ра, [*„-']„,, Q6Г), C33)
which is a natural fibre bundle over Mt. Let (Л, /,,..., /N) be a local
system of coordinates опЛ/, such that for the function A(Q) with fixed
/, parametrizes some domain of the curve Г - Г(/). Any such system
of coordinates will be called a local connection of the bundle Mt -*■ Mt
since for any path /(r) on Mt and for any point Q e ГA(т^), one can
locally define the lifting of the path onto Mt by defining the points Q(t)
e ГA(т)) by the condition A(Q(r)) = A(Q0).
The multivalued functions p, E, and О defined on each curve are
multivalued functions on Aft, i.e., p - p{\, I),E = £(A, /), and Q =
fl(A,7). If/depends ovlX, Y, and T, thenp, E, and Care functions of
A, X, Y, and T.
Theorem 17 ([HJ) For the asymptotic solution C20) with the
leading term of the form C29)-C31) and with bounded terms 1, and A,
to exist it is necessary that the equations
3p_CE_ _ d£\ _ dE_(Jp_ _ d£\
д\\~дТ Ty) 1\\ дТ дХ/
ЪО_1_Ьр_ _ ЪЕ\ _ <.ф+К
ЭЛ\ 3Y ЪХ) ~ (ф+Ф
dp
dA
C34)
be satisfied.
Equations C34) can be represented in an invariant form, which is
independent of the choice of the local connection A. If/depends on X,
Y, and T, then the inverse image of I(X, Y, T) in &г is a four-
dimensional manifold JC*dMv We consider the 1-form ы = pdX +
Ed Y + QdToviJi*. Then Equations C34) with JtaO are equivalent to
the condition that the external square of do» (which is a 4-form) should
be equal to zero on JCA, i.e.
do» Л do» = 0. C35)
The construction of solutions of Equation A) presented above
contains as a special case the construction of solutions of the Lax

82 I.M. KRICHEVER
equations L, = [A, L], We consider the submanifold M% С Mt
consisting of those data C24) for which the corresponding differential
d£ is exact, i.e. E - E(Q) is a single-valued function on Г. In this case
the coefficients of L and A are independent of у and A) is replaced by
the Lax equation. The function E(Q) can be used as the local
connection. In this case p - p(E, X, T), Q = Q(E, X, T), and
Equation C34) is transformed into
For kmO, Equation C36) is identical to the equation dTp = d^Q, which
was first obtained in [42] as a consequence of the averaged con-
conservation laws in the special case of the KdV equation.
3.3. Quasiclassical Limit of Two-Dimensional Integrable
Equations. Khokhlov-Zabolotskaya Equation
The 'null-zone' solutions, which correspond to curves Г of genus g=0
in our construction, constitute a class of simple solutions of the non-
nonlinear equations D). These solutions are of the form C27) and C28)
with fl, and vs being constant matrices. It turns out that the Whitham
equations are non-trivial even in this case, and, as will be seen later, in a
number of cases they are physically interesting in their own right. The
equations are identical with the quasiclassical limit of A). It follows
from the results of part 2 of this section that the equations can be
represented in the form
Л-^\^Е.- (^В.-^\Л+ (?E.-f¥l\f!£=n
dt ду/дк \dt дх/дк \ду дх)дк '
where p = p(k, x, y, t), E - E(k, x, y, t), and О - O(k, x, y, t) are
rational functions of k.
Example I Let/7 = k, E = а''(к~2-и), and О = к3 - ±uk - w. In
this case Equation B72) is equivalent to the system .
w* = а\щ, awy = ut + \uux. C38)
By eliminating w from C38), we obtain the Khokhlov-Zabolotskaya
equation.

PERTURBATION THEORY 83
Example 2 In the case where p = k,
Equation C37) leads to the system
(vA-Iv-fib-ых = 0, ч„-2@14^=0,
C39)
C40)
"«-"у-
The solutions of C40) that are independent of у correspond to the
classical limit of the non-linear vector Schrodinger equation. As was
first noted in [43], this equation describes TV-layer solutions of the
Benney equation. In [43] the quasiclassical limits of general Lax
equations were considered, and it was shown that they constitute the
compatibility condition for an algebraic and an ordinary differential
equation. As a result, a construction of the integrals of these equations
followed. The question concerning the construction of solutions
remained open. A scheme for solving the Cauchy problem for system
C40) based on developing the ideas of [43] was presented in [44]. Let us
note that the scheme can be easily obtained as a special case of our con-
construction of solutions of equations C37), which follows from the
results of the previous section.
In [14] (see also [9]) the present author gave a construction of exact
solutions of the Whitham equations C34) with AT=O. In one spatial
dimension the approach makes it possible to construct the solutions of
the Cauchy problem.
Let us present briefly this construction applied to the construction of
solutions of Equations C38) and C39).
We fix an arbitrary number N and we consider a polynomial of
degree N
X(k) = kN - — ukN-* - j w*N-3 + a4kN-4 + ... + %. C41)
The leading coefficients of the polynomial are uniquely defined by the
condition
p(k) = AI/N + O{k~l), E = A2™ + O{k~\ Q . A3/N

84 l.M. KR1CHEVER
where p, E, and 1? are the same as in Example 1.
We chose an arbitrary contour ^ in the Аг-plane and a smooth
differential dh(r) on ^. Having defined £(r) by the equality
A(f) = МФ(т)),
where ф(т) is a parametrization of,% one can locally deform the
contour % with the aid of A. We set
This function depends on the quantities (и, w, o4,. . ., eN), which play
the role of parameters, i.e.
\и, w,a4 aN).
We denote by ga,. . ., #N_2 the zeros of the differential dA:
d\(qj = 0. C43)
Locally, the parameters of J*" are uniquely defined by a system of
N-1 equations such that each of the equations is either of the form
- '
BW?, -u)y + C?,2 - \ u)t = 0, C44')
(qj+x +
OK
or of the form
4 - const. C44")
In particular, the system defines и and w as functions of the variables x,
y, and t, on which Equations C44) depend. (We stress once more that
the choice of Equations C44') and C44") to be included in the system is
arbitrary.)
Theorem 18 [14]. The functions u(x, t) and w(x, y, t) defined by the
system of equations C44) are solutions of the Khokhlov-Zabolotskaya
equation A0).
Note. The solution obtained within the framework of the scheme of
[45] is a partial case of the solutions constructed. )
To obtain the solutions of Equation C40) that are independent ofy,
we shall proceed as follows. We define .F by C42), where A = E(k) is
such as in Example 2 and $ is defined by the relation A(£) = ф (Т) (Ф is a
parametrization of the contour ^). The function •£*"(& |q,, oj depends

PERTURBATION THEORY 85
on r/, and j»i( which act as parameters. If the functions щ(х, t) and щ(х, t)
are defined by the system of equations
-rr-(Kjk, v$+x+ Щ = 0,j= 1,. . ., 2N,
OK
where /Cj are the roots of the equation
then they satisfy C40).
4. SPECTRAL THEORY OF TWO-DIMENSIONAL PERIODIC
SCHRODINGER OPERATORS
The main purpose of this section is to construct the spectral theory of
the operator
H=-dl-d$ + u(.x,y) C45)
with a smooth periodic potential u. It follows from the results of [20]
that the Floquet spectral set M1 С С3 (defined as the set of triplets of
complex numbers such that the equation
Щ{х,у, ty Q) = Еф(х,у, t, Q), Q = (E, wt, wj C46)
has a Bloch solution ф(х,у, t, Q), where Q С М1, with 'multipliers' w{
and W2) is an analytic submanifold in C3. The intersection of M1 С С3
and the hyperplane E = £"„ is called the complex Fermi curve Г6 cor-
corresponding to 'an energy level E'o'. As in the case of the operator A3),
the explicit construction of ГЕ and the resulting detailed description of
the structure of this_Riemann surface are based on a construction
carried out with the aid of the theory of perturbations of formal Bloch
solutions of Equation C46).
4.1. Perturbation Theory for Formal Bloch Solutions
Let u^x,y) be an arbitrary smooth periodic potential. We fix a
complex number w,0. A family of solutions ф,(х, у) of the equation
(- д\ - Ь) + ио(дг, у))ф, = 0 C47)

84 I.M. KRICHEVER
where p, E, and Q are the same as in Example 1.
We chose an arbitrary contour % in the Аг-plane and a smooth
differential dh(r) on <g. Having defined £(т) by the equality
where ф(т) is a parametrization of ^, one can locally deform the
contour % with the aid of A. We set
This function depends on the quantities (и, w,a4,..., aN), which play
the role of parameters, i.e.
w,a4, ...,oN).
We denote by q0 gN_2 the zeros of the differential dA:
dA(<?,) = 0. C43)
Locally, the parameters of J*" are uniquely defined by a system of
N-1 equations such that each of the equations is either of the form
Ctf-f u)t = 0, C44')
(<?,) + x + a
OK
or of the form
C\ - const. C44")
In particular, the system defines и and was functions of the variables x,
y, and t, on which Equations C44) depend. (We stress once more that
the choice of Equations C44') and C44") to be included in the system is
arbitrary.)
Theorem 18 [14]. The functions u(x, t) and w(x, y, t) defined by the
system of equations C44) are solutions of the Khokhlov-Zabolotskaya
equation A0).
Note. The solution obtained within the framework of the scheme of
[45] is a partial case of the solutions constructed. \
To obtain the solutions of Equation C40) that are independent of y,
we shall proceed as follows. We define J*"by C42), where A = E(k) is
such as in Example 2 and £ is defined by the relation A(£) = ф(Т) (ф is a
parametrization of the contour <g). The function 5F(k\i\Xi од depends

PERTURBATION THEORY 85
on щ and oif which act as parameters. If the functions ъ(х, t) and щ(х, t)
are defined by the system of equations
-тг(к>\т> »i) + x + 2/kj = 0,y= 1, . . ., 2N,
OK
where к, are the roots of the equation
then they satisfy C40).
4. SPECTRAL THEORY OF TWO-DIMENSIONAL PERIODIC
SCHRODINGER OPERATORS
The main purpose of this section is to construct the spectral theory of
the operator
H = -Э* - d1 + u(x,y) C45)
with a smooth periodic potential u. It follows from the results of [20]
that the Floquet spectral set M2 С С3 (defined as the set of triplets of
complex numbers such that the equation
Нф(х,у, t, Q) = Еф(х,у, t, Q), Q = (E, wlt wj C46)
has a Bloch solution ф(х, у, t, Q), where Q С М1, with 'multipliers' wx
and wj is an analytic submanifold in C3. The intersection of M1 С С3
and the hyperplane E = £"„ is called the complex Fermi curve Г6 cor-
corresponding to 'an energy level E'o\ As in the case of the operator A3),
the explicit construction of ГЕ and the resulting detailed description of
the structure of this, Riemann surface are based on a construction
carried out with the aid of the theory of perturbations of formal Bloch
solutions of Equation C46).
4.1. Perturbation Theory for Formal Bloch Solutions
Let и<Лх,у) be an arbitrary smooth periodic potential. We fix a
complex number w10. A family of solutions ф,(х, у) of the equation
( - dl - д2 + ио(х, у))ф, = О C47)

oo I.M. KR1CHEVER
is said to be basic if
Ых+К.У) = *юФ,(х,У); ФЛх.У+Ц = wMx.y) C48)
and if the following conditions are met:
1. There is a 'dual' family of solutions ф* (x,y)of the same equation
such that
< ФЖ - WA = T.&., ,т,Ф0. C50)
(Since ф, and ф+ satisfy C47)-C49), т. is independent of y.)
2. For any continuously differentiable function/(x) such that
/(*+/,)= wl0/(x), C51)
the series C52) and C53) converge to the following limits:
^. C52)
Example Let u0 - 4. Then for any k, each of the functions
ф(х,у, k) = exp^k+jjx + i(*-fyy) C54)
is a Bloch solution of C47) with the multipliers
Wl(k) = exp ( (*+^) 'i)! »iV<) = «p (l (k-£j l2). C55)
It can be directly verified that for any
w10 = w.(*o) * exp(±2/,), C56)
the sequence \
Ф,(х,У) = Их,У,к,) C57)
is a basic one. Here the numbers k, can be determined from the
equation vfio(£,) = wl0 and are equal to

PERTURBATION THEORY 87
-l <358>
(each of the indices v of k, is a pair (л, ±) consisting of an integral
number and a sign). The functions
Ф:(х,у) = ф(х,у, -к,) C59)
form the dual family.
Note The family of basic functions is, by definition, 'overdeter-
mined', and sof(x) cannot be uniquely expanded in terms of ф, or ф„.
At the same time, for any couple of functions/(дг) and g(x) that satisfy
C51), there are unique constants c,(y) such that
/(*) - S MjOiM*.УУ- Six) = S 'ЛуЖОс.У)- C60)
It follows from C50) that the constants are equal to
c, = ^' ~g^\ C61)
r.
We denote by '0' any one of the indices v and we shall assume that
wto * wb> v Ф 0. C62)
Lemma 23 If condition C62) is satisfied, then for any continuously
dif ferentiable function 6u (x, y) (with the same periods as uo(x, y)) there
are unique formal series
QJ>, C63)
5-1
, у, Q) - 2 Ф,(х, у, Ш. Фо - W^. J-). C64)
1-0
such that the equation
(-ax2-ay2+M0 + 5u)*- = 2F*y + (Fy + F2)TP C65)
and the conditions
/„ J-, С) - wl0nx,y, Q),
t Q) ш Wn*{x,y,Q),

88 I.M. KR1CHEVER
= Ч = ^о,П ...„,._
C67)
hold (for the time being, Qo denotes the pair (и»,,,, jvm)).
Proof Equation C65) is equivalent to the system
* /
C68)
We shall seek solutions of C68) in the form
*.5»2<SOW.Cx,.y). C69)
assuming that c,' are chosen in such a way that
с,у). C70)
It follows from the note above that this can be done in a unique way.
From C69) and C70) it follows that
Т,с'„Ф, = 0. C71)
Substituting C70) and C69) into C68), we find that
where R, is the right-hand side of C68). From C71) and C72) it follows
that
c%= <RM\t;1. C73)
These equations, combined with the condition
с%у + Ц~~сЦу), C74)
enable us to define c\ uniquely for v Ф 0. Condition C67) is sufficient

PERTURBATION THEORY 89
for a periodic solution of C73) to exist for v = 0. The condition defines
F, uniquely. The final formulae have the form
Ft = т.-Чч
co°= 1, c0' = -т0-' 2 Ъ<Ф,-№>„ О 1. C76)
/»i
c° = 0 for v Ф 0, and for j ^ 1 we have
T
C77)
Corollary The formula
Й*„>\ Go) - exp/ jF(y, G0)d7' J *(дг,^ Qe)*-'@, 0, Q0) C78)
defines a formal Bloch solution of the equation
$ й 0,и = ио + &и, C79)
4>{x+h,y, Go) = ^(л-,^, Q^; Ф(х,у+12, Go) = «^(дг,у, Go).
C80)
where
'i
', Q0)dy'\ . C81)
/
We handle the resonance case (i.e. in the case where condition C62)
does not hold) in complete analogy with what is done in Section 2. We
denote by / an arbitrary finite subset of indices v such that
Уъ. * W2,> « 6/, к*/. C82)

90 I.M. KRICHEVER
Lemma 24 There exists a unique matrix-valued formal series
Ш
F(y, ww) = 2 F,{y, wi9), F = (/?), a, /3 € / C83)
such that Equation C65) (where Fis now a matrix and if'is a vector) has
a formal solution У whose components satisfy the conditions
,y, wl0);
—- C84)
, »v10) = и^ *«(jf, .у, »v10);
C85)
The proof of the lemma is analogous to that of Lemma 23. For the sake
of conciseness, we omit the corresponding recurrent formulae for the
coefficients F, and c*-a since they are exact matrix analogues of the
formulae for the non-resonance case.
We define the matrix T(y, wl0) by the equation
dy T + TF = 0, 27@, w10) = 6fl". C86)
Then each of the components & of Ф = Г* is a solution of C79). As in
Section 1, we can prove that the assertion of the corollary of Lemma
9 holds, that is, to each point of the surface given by the charac-
characteristic Equation B37) there corresponds a unique Bloch solution ф of
Equation C79).
Note All the assertions of Section 2.2 concerning the construction
of the 'dual' functions ф*(х,у, Q^, which are defined on the same
surfaces as ${x, y, Q), can also be carried over to the case in question.
4.2. Structure of Complex 'Fermi Curves'
Let но = 4. Then, as already mentioned, Equation C47) has a basic
sequence of Bloch solutions for any jv10 ф e±3it. Therefore, the
formulae of Lemma 23 define formal Bloch solutions ф(х,у, к^ o,f
Equation C46) if we set 6м = и - E - 4 and if k0 satisfies condition
C62), which ensures that there are no resonances. It follows from C54)
that the resonance pairs of points have the form (k^M, Ar^M) and (££м,
£nm). where

PERTURBATION THEORY 91
2), C87)
2), C88)
Znm = ^r + ^r-.N.MeZ. C89)
111 *<2
The set of such points has only two points of accumulation, namely
k = 0 and k=oo.
The following constructions and assertions are practically the same
as their analogues in Section 2.3. Therefore, we restrict ourselves to
brief formulations in which we indicate, as far as it is necessary, the
slight changes which should be introduced in the proofs and
constructions of Section 2.3.
Having fixed h, one can choose the neighbourhoods R^ and RfjM of
the resonance points C86) and C87) in such a way that the inequalities
I *-nwV - 11 > Л, | и'й'и'г,- 11 > h C90)
hold for any k0 that does not belong to these neighbourhoods. It can be
assumed that h is sufficiently small so that the neighbourhoods do not
intersect each other. We assume that the periodic function u(x,y)
admits analytic continuation to a neighbourhood of the real values x,y
(i.e. the function satisfies B47) for some U, т,, and tJ.
Lemma 25 There is a constant No such that for any k0 that does not
belong to Rwt or R^ and satisfies the condition \ko\ + |k^' | > No,
the perturbation series constructed by virtue of Lemma 7 (for ио=4
and 8u = и - E - 4) and the corollary of that lemma, converges
absolutely and uniformly, and defines a Bloch solution ф(х,у, £„) of
Equation C46), which is analytic (with respect to x, y, and £„) and does
not vanish.
Note In complete analogy with the previous construction, one can
set up the perturbation series for the formally adjoint function
Ф(х,у, к0), which is analytic in the non-resonance domain just as ф is.
Now, we consider k0 e R£M (or R£M) such that \ko\ + |kg11 > No.
As the set of resonance indices we choose v = Q and p = v0 such that
**6^nm (°r ^nm> respectively). Then for wl0 e W^^ = w{(R^M) (or
^nm = w\(rnm)> 'he perturbation series in Lemma 8 define a two-
dimensional quasi-Bloch solution of Equation C46). The cor-
corresponding monodromy matrix f= ТAг, wl0) defines a two-sheet
covering J?NM or А~ш over the domains WUM and Й^. Again, we say

92 . I.M. KRICHEVER
that a pair N, Mis distinguished if the discriminant of the characteristic
equation for /'has a double zero.
Lemma 26 For any pair N, M that is.not distinguished, the Bloch
function ф admits continuation to /?NM №NM), where it has one simple
pole.
Lemma 27 Let ф(х,у, Q) and ф*(х,у, Q) be Bloch solutions of
C46), where Q is a non-singular point on the surface ГЕ. Then the
equality
4A Ш+-Ф;Ф>у + <fc,<W+ - W;>» - 0 C91)
holds. There are no common zeros of the functions < ф^ф+ - фф* >у and
<.фуф* - ффу >х in the non-singular domain ГЕ.
Equality C91) can be proved in the same way as A64). The second
assertion of the lemma follows from the fact that
* - фф: >у+№р,<фгф+ - фф; >х=
C92)
for the variation 5и of the potential и of the operator C44).
By analogy with Section 2, we construct the continuation of
$- (дг, y, k9) to the interior of the 'central resonance domain' Ro such that
I *o I + I *o I ~' < ^o» which is replaced by the finite-sheeted covering £0
of the domain 1VO = w,(/?o).
We denote by ГЕ the Riemann surface obtained by 'pasting in' /?NM
and ^NM in place of some excised neighbourhoods of the resonance
points that are not distinguished and by 'pasting in' /?0 in place of
Theorem 19 The Riemann surface FE is isomorphic with the
'spectral Fermi curve' of the operator C45). Each Bloch solution
ф(х, у, Q), Q e ГЕ of this equation normalized by the condition ф@,0,
Q) ж 1 is meromorphic on ГЕ. The poles of ф are independent of x, y.
The function has one simple pole both in i?^ and in J^ (N, Mis a pair
that is not distinguished), and it has g0 poles in /?0, where g0 is equal to
the genus of Ro in the general situation of non-singular /?„. ф is
holomorphic outside /?0, /?NM, and Лмм.
All the assertions of the theorem except the first follow from th^
construction of ГЕ only. To each point Q of the surface ГЕ there
correspond the multipliers w,(Q), i = 1,2, which define a mapping from
Гв into the corresponding 'Fermi curve'. The fact that the mapping
establishes an isomorphism follows from the lemma below.

PERTURBATION THEORY 93
For any complex number и>,0, we denote by Q, e Ге the solutions of
the equation
",«2.) = и-,,, C93)
and we denote by ф,(х,у) the functions Ф(х,у, Q,).
Lemma 28 If Equation C93) has simple roots, then the family of
functions ф,{х,у) is a basic one (in the sense of the definition given at
the beginning of part 1 of this section).
Proof It follows from Lemma 27 that the differential
C94)
is hoiomorphic on Гв and has zeros at the points where ф and ф* have
poles. The assertion of the lemma can be derived by considering the
contour integrals
51N = ] d,
с„ о
I,
1 - wiOw,
См О
where CN is the union of two contours that encompass the points, have
radii of order N and ЛГ1, and do not pass through the resonance
domains. The integrals tend to zero and tof(x), respectively, as iV-*oo,
Since the residues of the integrands coincide with the terms of the series
C52) and C53), the lemma is proved.
Corollary 1 The correspondence
Ти-,, wO-*V¥i\ Wj-') C97)
defines a hoiomorphic involution а: Гв -» Гв of Fermi curves.
Proof To each point Q e Гв there corresponds a Bloch solution
ф{х,у,О) with multipliers w,«2) and w2(Q), and a 'dual' function
Ф*(х,У, Q) with multipliers wf'(B) and v?i\Q)< Since ф* is a Bloch
solution of the same equation C45) and the points of ГЕ parametrize all
Bloch solutions, it follows that the pair w," 1{Q), и>2~ \Q) belongs to the
Fermi curve. The lemma is proved. At the same time, we find that

94 I.M. KRICHEVER
ФЧх, У, Q) = Ф(х, у, c(Q)). C98)
Corollary 2 If the potential u(x,y) is real-valued, then the anti-
hoiomorphic involution т induced by the mapping
(и>„ wji - (w,, v?j) C99)
is defined on Гв. In this case
Их,у, Q) = ф(х,у, t(Q)). D00)
Definition We say thatu is a 'finite-zone potential with respect to a
level £У if all but a finite number of pairs N, Mate distinguished for
the construction of Ги, i.e. if Г^ is of finite genus.
It follows from the definition of distinguished pairs that for 'finite-
zone potentials with respect to a level Eo\ the surface Г^ outside a
bounded domain \ko\ + \ko\~l < Af, coincides with some neigh-
neighbourhoods of the points k = 0 and fc=» on the ordinary complex
plane. Therefore, the surface admits compactification by two
'infinitely distant' points P±. In what follows we retain the symbol F^
to denote the corresponding compact Riemann surface.
Theorem 20 For finite-zone potentials и with respect to Eo, the
Bloch solutions of Equation C46) with E=E0 are defined outside the
set consisting of the two points P± of the compact Riemann surface Г^,
on which there exists a holomorphic involution <r: Г^ -♦ Ги. In a
neighbourhood of P± the function ф(х,у, Q), Q e Г^ has the form
Ф(х,У, Q) = exp((x±iy)k±)l 1 + 2 **(x,y)*;1), D01)
where £"'• = Л^Чб) are local parameters (such that k±(o(Q))
ш neighbourhoods of P±. ф is a meromorphic function
outside the set of points P±. It has g poles, which are independent of x,
y, where g is the genus of Г^ in the general situation of non-singular
Ги. In this case the poles 7, and 7,* = o(yj are zeros of the differential
of the third kind dO, which has simple poles at P± and is holomorphic
everywhere except at P±. If u(x, y) is real-valued, then there is an anti-
holomorphic involution т on JT^ which commutes with a, such ihat
t(P±) = PTu.ndk±(j(Q)) = k*(Q). Moreover, the set of poles of ф is
invariant with respect to r.
The proof of the following theorem is completely analogous to that
of A02).

PERTURBATION THEORY 95
Theorem 21 For any Eo, each smooth periodic potential u{x,y) of
the operator C41) that admits analytic continuation to some neigh-
neighbourhood of the real variables x, у can be uniformly approximated
along with any number of derivatives by 'finite-zone potentials ua(x, y)
with respect to the level Eo'.
4.3. 'Finite-Zone with Respect to a Level Eo' Two-Dimensional
Periodic Schrddinger Operators
An important difference between the spectral theory of non-stationary
Schrddinger operators A3) with <r=l and two-dimensional
Schrddinger operators with real-valued smooth periodic potentials
u(x,y) consists in that in the former case the corresponding spectral
curve Г is always non-singular, while in the latter case the 'complex
Fermi curve' Г^ can have a finite number of singular points. The
complete description of possible types of singularities has not so far
been found.
We start this part of the paper with a short presentation of the
inverse problem of the reconstruction of 'finite-zone with respect to a
level Eo' potentials u(x, y) in the case of non-singular 'Fermi curves' Ги
([22], [23]).
Let Г be a non-singular algebraic curve of genus g with two distin-
distinguished points P± and with local parameters k'±\Q) such that
*±'CP±) = O chosen in some neighbourhoods of these points. For any
sequence of g points 7,, . . ., yf in general position, there is a unique
Baker-Akhiezer function ф(х,у, Q), which is meromorphic on Г
except at P±, has poles at the points 7,, and exhibits the following
asymptotic behavior:
-А , k± = k±{Q), Q-P±, D02)
ф = ek-*c(x,y)( 1 + S £"<». >)*:') ,z = x + iy,z = x- iy.
It was proved in [21] that such a function ф satisfies the equation
0,Й = -82п + А& + и, D04)

96 I.M. KRICHEVER
where
) m dzlnc{x,y), u(x,y) = d-zSt{x,y). D05)
Explicit formulae involving theta functions were obtained for Ф, and
for /lj and м, respectively.
In [22,23] sufficient conditions were found which should be satisfied
by the data (Г, P±,k±, y^ in order that the corresponding operator Й
be a purely potential operator, i.e. Az = 0. These are the following
conditions:
(i) there is an involution а: Г-* Г on Г with two invariant points
P±
(ii) the local parameters A:;1 should satisfy the condition k±(a(Q))
= -k±(Q)
(iii) the points yt and y* = a(y,) form a divisor of zeros of the
differential of the third kind dtf with unique simple poles at P±.
The sufficiency of these conditions follows from the fact that if the
conditions are satisfied, then the differential
dfi - ф(х,у, Q)V(x,y, Q)dO(Q), Г = Ф(х,у, a(Q)) D06)
is holomorphic on Г except at Pt, where it has simple poles. Since the
sum of residues of this differential is equal to zero, it follows that c2=s 1
(since c@,0)= 1, it follows that c(x,y)*s 1). This is sufficient for the
equality Лг = 0 to hold.
Theorem 22 The above conditions ((i)-(iii)) for the data (Г, P±, к ±,
7,) of the inverse problem are necessary for the corresponding operator
D04) to be a potential operator (i.e. to have the form C46)) and for the
potential u(x,y) to be smooth. If и is periodic, then Г is isomorphic
with the 'complex Fermi curve' ГЕ-о-
Proof In the general case the operator H that corresponds to the
data (Г,Р±,к±, у^ is quasiperiodic. The periodicity conditions can be
stated in exactly the same way as for the case of finite-zone non-
stationary Schrddinger operators. We define the differentials d^ arid
dpy of the quasimomenta as the differentials of the second kind on Г
that have unique poles of the form \
dA = -idk±(l+O(K2)), d>y= ±dk±(l + O(k-2)) D07)
at the points P± and are uniquely normalized by the conditions
ensuring that their periods with respect to each of the cycles on Г are

PERTURBATION THEORY 97
real. If the periods are divisible by 2т//, for dp, and by 2т//2 for dpy,
then Й has periods /, and 1г with respect to x and y, respectively. For
periodic potential operators, the last assertion of the theorem can be
proved in exactly the same way as the first assertion of Theorem 19.
Then the necessity of conditions (i)-(iii) for periodic operators follows
from Theorem 20. The real matrices formed by the periods of the
differentials dpx and dpy are non-degenerated functions of the
parameters (Г, P±, [A:;1],). Therefore, as /„ /2 ~* oo, the set of periodic
operators is dense among all finite-zone operators with respect to a
fixed level (corresponding to smooth curves). This enables us to close
the proof of the theorem.
In the same way one can prove that for u(x, y) to be real-valued it is
necessary that there be an anti-involution т on Г such that т(Р±) - PT,
k+{r(Q) " £-«2). and that the divisor of poles 7,,. . .,7, be invariant
with respect to т. The involution a and the anti-involution т commute
with each other.
In [23] sufficient conditions were formulated which should be
satisfied by the parameters (Г, a, r, P±1 k±, yj in order that the cor-
corresponding potential и of the operator C46) be smooth. Apart from
these conditions, it is sufficient that Г be an M-curve with respect to т
and that among the ovals aQ, a,,. . ., at that are invariant with respect
to т there be g ovals such that a(a}) - ab+i. Here g0 is the genus of the
curve Г/а. Since a has two invariant points, it follows that g = 2g0 for
/= 1 g0. If the points 7, are chosen in such a way that there is one
of the points on each of the ovals at, s = 1,. . ., g, then the correspond-
corresponding potential u is smooth.
Apart from these conditions, there is also another type of sufficient
conditions. If the anti-involution та is of dividing type and the dif-
differential dflis positive on each of the invariant ovals of та with respect
to the orientation defined on the ovals by regarding them as the
boundary of one of the domains obtained as a result of dividing Г by
the ovals, then и is smooth.
These two types of sufficient conditions are analogous to the
conditions which ensure that the finite-zone potentials for the operator
A3) with a = 1 and 0 = i, respectively, are smooth. The proofs of these
assertions are also completely analogous (see [27]).
In a recent paper [46] a whole range of sufficient conditions were
found, among which the conditions mentioned in the present paper
occupy entirely different positions. The method used in [46] is based on

i.m. KRICHEVER
the analysis of the formulae for u(x,y) involving theta functions, and
differs fundamentally from the approach developed in the present
paper. Not all of the conditions given in [46] have yet been recast in the
form needed in our approach. As shown in [46], the conditions are not
only sufficient but also necessary for the potentials и corresponding to
smooth curves ГЕ_0 to be smooth themselves. The potentials have the
form
u(x, y)=- 2дгд-г In 6(Utz + U2z + f) + c, D08)
where the constant с depends on Г and P± (the explicit form of the
constant was found in [47]), and the theta function в is the Prym theta
function, i.e. it is constructed from the matrix of periods of hoio-
morphic differentials that are odd with respect to a. For certain types
of degenerations of Г, the Prymian of the curve can remain non-
degenerated (in contrast to the Jacobian, which is always degenerated).
This feature is what calls forth the possibility that there are smooth
quasiperiodic finite-zone potentials corresponding to singular curves.
The most interesting case, which yields the ground state of H, was
presented in [23, 48]. One can construct more general examples using
the well-known technique for constructing multisoliton solutions with
finite-zone background (for the case of operators of the type A3), see
[27]). We omit the detailed description of these examples, because the
complete description of admissible types of degenerations is unknown
to us at the present time. To find the answer to this question, one needs
to carry out a more detailed investigation of the direct spectral problem
considered in the previous part of this section.
Note that the related question concerning the description of possible
types of degenerations is discussed in an article by Shiota included at
the end of the Russian edition of the book [49].
One can see from the results of the previous part of this section that
the set of potentials corresponding to smooth curves, i.e. potentials of
the form D08), is dense among all finite-zone potentials (cor-
(corresponding to curves which may have singularities). Therefore, the
theorem asserting that the set of finite-zone potentials is dense means
that the set of potentials of the form D08) is dense too. \
Finally, note that the limited length of this paper forces us to omit a
discussion of the applications of the spectral theory of two-
dimensional periodic Schrddinger operators to the theory on nonlinear
equations. The construction of the theory of perturbations of periodic

PERTURBATION THEORY 99
solutions of the Novikov-Veselov equation and the derivation of the
Whitham equations for these solutions (which, incidentally, have the
same form as C24) after the substitution dp=dpx, dE=dpr) are
completely analogous to the constructions in Section 3. In analogy with
Section 2.2, one can prove the completeness of the products of the
Bioch functions ф and ф* at the resonance points and the products
Ф (x, y, Q№*(x, y, Q) in the space of periodic functions with respect to
x and y, along with a number of other assertions.
REFERENCES
1. Krichever, I.M. A976) An algebraic-geometric construction of the
Zakharov-Shabat equations and their periodic solutions, Dokl. Akad. Nauk SSSR,
227, No. 2, 291-294.
2. Krichever, I.M. A977) Integration of non-linear equations by the methods of
algebraic geometry, Funktsional. Anal, i Prilozhen., 2, No. 1, 15-31.
3. Krichever, I.M. A977) The methods of algebraic geometry in the theory of non-
nonlinear equations, UspekhiMat. Nauk, 32. No. 6, 180-208.
4. Dubrovin, B.A., Matveev, V.B. and Novikov, S.P. A976) Non-linear equations of
the Korteweg-de Vries type, finite-zone linear operators, and Abelian varieties,
UspekhiMat. Nauk, 31, No. 1, 55-136.
5. Zakharov, V.E., Manakov, S.V., Novikov, S.P. and Pitaevskii, L.P. A980) The
theory ofsoiitons: the inverse problem method. Nauka, Moscow.
6. Zakharov, V.E. and Shabat, A.B. A974) Integration of equations of mathematical
physics by the method of the inverse problem of scattering theory. I, Funktsional.
Anal, iPrilozhen., 8, No. 3, 43-53.
7. Dryuma, V.S. A974) Ал analytic solution of the two-dimensional Korteweg-de
Vries equation, Pis'ma Zh. Eksper. Teoret. Fit., 19, No. 12, 219-225.
8. Zakharov, V.E. and Shulman, E.I. A985) Integrability problems for spatially two-
dimensional systems, Dokl. Akad. Nauk SSSR, 283, No. 6, 1325-1329.
9. Krichever, I.M. A989) The spectral theory of two-dimensional periodic operators
and applications, Uspekhi Mat. Nauk, 44. No. 2, 121-184.
10. Krichever, I.M. A988) The periodic problem for the KP-2 equation, Dokl. Akad.
Nauk SSSR, 298, No. 4, 802-806.
11. Krichever, I.M. and Grinevich, P.G. A989) Algebraic geometry methods in so/iton
theory, Physica D.
12. Doorokhotov, S.Yu. and Maslov, V.P. A980) Finite-zone almost periodic
solutions in WKB approximations. In Progress in Science and Technology, Current
Problems in mathematics, 3-94. Vsesoyuz. Inst. Nauchn. iTekhn. Inform., Akad.
Nauk SSSR, Moscow.
13. Dobrokhotov, S.Yu. and Maslov, V.P. A982) Multiphase asymptotics of non-
nonlinear partial differential equations with a small parameter, Sov. Sci. Rev., Math.
Phys.. Vol. 3,221-280.
14. Krichever, I.M. A988) The averaging method for two-dimensional 'integrable'
equations, Funktsional. Anal, i Prilozhen., 22, No. 3, 37-52.
15. Tsarev, S.P. A985) The Poisson brackets and one-dimensional Hamiltonian
systems of the hydrodynamic type, Dokl. Akad. Nauk SSSR, 283, No. 3, 534-537.

i.M. KRICHEVER
the analysis of the formulae for u{x,y) involving theta functions, and
differs fundamentally from the approach developed in the present
paper. Not all of the conditions given in [46] have yet been recast in the
form needed in our approach. As shown in [46], the conditions are not
only sufficient but also necessary for the potentials и corresponding to
smooth curves ГЕ_0 to be smooth themselves. The potentials have the
form
u(x,y) = -23t3£ln в(С/,г + U2z + ?) + c, D08)
where the constant с depends on Г and P± (the explicit form of the
constant was found in [47]), and the theta function в is the Prym theta
function, i.e. it is constructed from the matrix of periods of holo-
morphic differentials that are odd with respect to a. For certain types
of degenerations of Г, the Prymian of the curve can remain non-
degenerated (in contrast to the Jacobian, which is always degenerated).
This feature is what calls forth the possibility that there are smooth
quasiperiodic finite-zone potentials corresponding to singular curves.
The most interesting case, which yields the ground state of H, was
presented in [23, 48]. One can construct more general examples using
the well-known technique for constructing multisoliton solutions with
finite-zone background (for the case of operators of the type A3), see
[27]). We omit the detailed description of these examples, because the
complete description of admissible types of degenerations is unknown
to us at the present time. To find the answer to this question, one needs
to carry out a more detailed investigation of the direct spectral problem
considered in the previous part of this section.
Note that the related question concerning the description of possible
types of degenerations is discussed in an article by Shiota included at
the end of the Russian edition of the book [49].
One can see from the results of the previous part of this section that
the set of potentials corresponding to smooth curves, i.e. potentials of
the form D08), is dense among all finite-zone potentials (cor-
(corresponding to curves which may have singularities). Therefore, the
theorem asserting that the set of finite-zone potentials is dense means
that the set of potentials of the form D08) is dense too. ^
Finally, note that the limited length of this paper forces us to omit a
discussion of the applications of the spectral theory of two-
dimensional periodic Schrddinger operators to the theory on nonlinear
equations. The construction of the theory of perturbations of periodic

PERTURBATION THEORY 99
solutions of the Novikov-Veseiov equation and the derivation of the
Whitham equations for these solutions (which, incidentally, have the
same form as C24) after the substitution &p=dpx, d£=dpr) are
completely analogous to the constructions in Section 3. In analogy with
Section 2.2, one can prove the completeness of the products of the
Bloch functions ф and ф* at the resonance points and the products
Ф(х, у, Q)$*{x,y, Q) in the space of periodic functions with respect to
x and y, along with a number of other assertions.
REFERENCES
1. Krichever, I.M. A976) An algebraic-geometric construction of the
Zakharov-Shabat equations and their periodic solutions, Dokl. Akad. Nauk SSSR,
227. No. 2, 291-294.
2. Krichever, I.M. A977) Integration of non-linear equations by the methods of
algebraic geometry, Funktsional. Anal. iPrilozhen., 2, No. 1, 15-31.
3. Krichever, I.M. A977) The methods of algebraic geometry in the theory of non-
nonlinear equations, Uspekhi Mat. Nauk. 32, No. 6, 180-208.
4. Dubrovin, B.A., Matveev, V.B. and Novikov, S.P. A976) Non-linear equations of
the Korteweg-de Vries type, finite-zone linear operators, and Abelian varieties,
Uspekhi Mat. Nauk, 31, No. I, 55-136.
5. Zakharov, V.E., Manakov, S.V., Novikov. S.P. and Pitaevskii, L.P. A980) The
theory o/solitons: the inverse problem method. Nauka, Moscow.
6. Zakharov, V.E. and Shabat, A.B. A974) Integration of equations of mathematical
physics by the method of the inverse problem of scattering theory. 1, Funktsional.
Anal. iPrilozhen., 8, No. 3, 43-53.
7. Dryuma, V.S. A974) An analytic solution of the two-dimensional Korteweg-de
Vries equation, Pis'ma Zh. Eksper. Teoret. Fiz., 19, No. 12, 219-225.
8. Zakharov, V.E. and Shulman, E.I. A985) Integrability problems for spatially two-
dimensional systems, Dokl. Akad. Nauk SSSR, 283, No. 6, 1325-1329.
9. Krichever, I.M. A989) The spectral theory of two-dimensional periodic operators
and applications, Uspekhi Mat. Nauk, 44, No. 2, 121-184.
10. Krichever, I.M. A988) The periodic problem for the KP-2 equation, Dokl. Akad.
Nauk SSSR, 298, No. 4, 802-806.
11. Krichever, I.M. and Grinevich, P.G. A989) Algebraic geometry methods in soliton
theory, Physica D.
12. Doorokhotov, S.Yu. and Maslov, V.P. A980) Finite-zone almost periodic
solutions in WKB approximations. In Progress in Science and Technology, Current
Problems in mathematics, 3-94. Vsesoyuz. Inst. Nauchn. i Tekhn.-Inform., Akad.
Nauk SSSR, Moscow.
13. Dobrokhotov, S.Yu. and Maslov, V.P. A982) Multiphase asymptotics of non-
nonlinear partial differential equations with a small parameter, Sov. Sci. Rev., Math.
Phys., Vol. 3, 221-280.
14. Krichever, I.M. A988) The averaging method for two-dimensional 'integrable'
equations, Funktsional. Anal. iPrilozhen., 22, No. 3, 37-52.
15. Tsarev, S.P. A985) The Poisson brackets and one-dimensional Hamiltonian
systems of the hydrodynamic type, Dokl. Akad. Nauk SSSR, 283, No. 3, 534-537.

100 I.M. KR1CHEVER
16. Dubrovin, B.A. and Novikov, S.P. A983) Hamiltonian formalism for one-
dimensional systems of the hydrodynamic type and the Bogolyubov-Whitham
averaging method, Dokl. Akad. Nauk SSSR, 270, No. 3, 781-785.
17. Dubrovin, B.A. and Novikov, C.P. A984) On the Poisson brackets of the
hydrodynamic type, Dokl. Akad. Nauk SSSR, 279, No. 2, 294-297.
18. Bakhyalov, N.S., Zhileikin, Ya.M. and Zabolotskaya, E.A. A982) Non-linear
theory of sound beams. Nauka. Moscow.
19. McKean, H. and Turbovitz, E. A977) Hill's operator and hyperelliptic functions
theory in the presence of infinitely many branch points, Camm. PureAppL Math.,
29, 143-226.
20. Kuchment, P.A. A982) The Floquet theory for partial differential equations,
Uspekhi Mat. Nauk, 37, No. 4, 3-52.
21. Dubrovin, B.A., Krichever, I.M. and Novikov, S.P. A976) The Schrodinger
equation in a periodic field and Riemann surfaces, Dokl. Akad. Nauk SSSR, 229,
No. 1, 15-18.
22. Veselov, A.P. and Novikov, S.P. A984) Finite-zone two-dimensional periodic
SchrOdinger operators: explicit formulae and evolution equations, Dokl. Akad.
Nauk SSSR, 279, No. 1, 20-24.
23. Veselov, A.P. and Novikov, S.P. A984) Finite-zone two-dimensional periodic
Schrodinger operators: the potential case, Dokl. Akad. Nauk SSSR, 279, No. 4,
784-788.
24. Krichever, l.M. A983) 'Hessians' of the integrals of the Korteweg-de Vries
equation and perturbations of finite-zone solutions, Dokl. Akad. Nauk SSSR, 270,
No. 6, 1312-1317.
25. Novikov, S.P. A974) A periodic problem for the Korteweg-de Vries equation.
Funktsional. Anal, iego Prilozhen., 8, No. 3, 54-66.
26. Us, A.R. and Matveev, V.B. A973) The SchrOdinger operators with a finite-zone
spectrum and the N-soliton solutions of the Korteweg-de Vries equation, Teoret.
Mat. Fiz.. 23, No. 1, 51-67.
27. Krichever, l.M. A986) The spectral theory of 'finite-zone' non-stationary
Schrodinger operators. The non-stationary Peierls method, Funktsional. Anal, i
ego Priiozhen., 20, No. 3, 42-54.
28. Dobrokhotov, S.Yu. A988) Resonances in the asymptotics of the solution of the
Cauchy problem for the Schrddinger equation with a rapidly oscillating finite-zone
potential. Mat. Zametki, 44, No. 3, 319-341.
29. Dobrokhotov, S.Yu. A988) The resonance correction to the adiabatic solution of
the Korteweg-de Vries equation. Mat. Zametki, 44, No. 4, 551-555.
30. Kuksin, S.B. A988) The theory of perturbations of conditionally periodic solutions
of infinite dimensional Hamiltonian systems and applications to the Korteweg-de
Vries equation. Mat. Sb., 136A79), No. 3, 396-412.
31. Kuksin, S.B. A989) Reducible variationat equations and perturbations of invariant
tori for Hamiltonian systems, Mat. Zametki, 45, No. 5, 38-49.
32. Kozel, V.A. and Kotlyarov, V.P. A976) Almost periodic solutions of the equation
ifo-uM = sin u, Dokl. Akad. Nauk Vkr. SSR Ser. A, 10, 878-881.
33. Its, A.R. Finite-zone solutions of the Sine-Gordon equation (see Matveev, V.B.
A976) Abelian functions and solitans. Preprint, Wroclaw University, N 373).
34. Dobrokhotov, S.Yu. and Maslov, V.P. A979) The problem of reflection off the
boundary for the equation A u+ashu=0 and finite-zone conditionally periodic
solutions, Funktsional. Anal, i ego Prilozhen., 13, No. 3, 79-80.
35. Forest, M.G. and McLaughlin, D.W. A983) Modulations and Sine-Gordon and
Sine-Gordon wavetrains. Stud. Appl. Math., 68, 1, 11-59.

PERTURBATION THEORY 101
36. Krichever, l.M. A983) Non-linear equations and elliptic curves. In: Progress in
Science and Technology, Current Problems in Mathematics, Vol. 23, 79-136.
Vsesoyuz. Inst. Nauchn. i Tekhn. Inform., Akad. Nauk SSSR, Moscow.
37. Dubrovin, B.A. A981) Theta functions and non-linear equations, Uspekhi Mat.
Nauk, 36, No. 2, 11-80.
38. Dubrovin, B.A. A983) Finite-zone matrix operators. In Progress in Science and
Technology, Current Problems in Mathematics, Vol. 23, 33-78. Vsesoyuz. Inst.
Nauchn. i Tekhn. Inform., Akad. Nauk SSSR, Moscow.
39. Dubrovin, B.A. and Natanzon, S.M. A988) Real theta-function solutions of the
Kadomtsev-Petviashvili equation, Izv. Akad. Nauk SSSR, Ser. Mat., 52, No. 2,
267-286.
40. Marchenko, V.A. A977) Sturm-Liouville operators and applications. Naukova
Dumka, Kiev.
41. Firsova, N.E. A975) The Riemann surface for the quasimomentum and scattering
theory for the perturbed Hill operator, Zap. Nauchn. Sem, Leningrad. Otdel. Mat.
Inst. Steklov. (LOMl), 51, No. 7, 183-196.
42. Flashka, H., Forest, M.G. and McLaughlin, D.W. A980) Multiphase averaging
and the inverse spectral solution of the Korteweg-de Vries equation, Comm. Pure
Appl. Math., 33, 739-784.
43. Zakharov, V.E. A980) Benney equations and quasiclassical approximation in the
inverse problem method, Funktsional. Anal, i ego Prilozhen., 14, No. 2, 15-24.
44. Geodzhaev, V.V. A988) The solution of the Benney equation, Teoret. Mat. Fit.,
73, No. 2, 225-263.
45. Kodama, Y. and Gibbons, 1. A989) A method for solving the dispersionless KP
hierarchy and its exact solutions, Phys. Lett. A, 135, No. 3, 167-170.
46. Natanzon, S.M. A988) Non-singular finite-zone two-dimensional Schrodinger
operators and Prymians of real curves, Funktsional. Anal, i ego Prilozhen., 22,
No. 1,79-80.
47. Taimanov, I.K. A986) Bloch eigenfunctions for some two-dimensional periodic
linear operators, Dokl. Akad. Nauk SSSR, 289, No. 5, 1653-1657.
48. Veselov, A.P., Krichever, l.M. and Novikov, S.P. A985) Two-dimensional
periodic SchrOdinger operators and Prym's theta functions, 'Geom. Today', Int.
Conf. Rome, June 4-11, 1984, Boston, 283-301.
49. Mumford, D. A983) Tata lectures on theta functions 1, 11, Boston, Basel.

INDEX
Periodic problem for KP-type equations
— perturbation theory for finite-zone
solutions of KP-2 73
— quasiclassical limit of two-
dimensional integrable equations.
Khokhlov-Zabolotskaya equation 82
— Whitham equations for spatially
two-dimensional integrable systems 78
Perturbation theory of finite-zone
solutions of evolution Lax-type
equations 8
— KdV equation 13
— Sine-Gordon equation 27
Spectral theory of non-stationary
Schrudinger operators
— finite-zone case 38
— perturbation theory for formal Bloch
solutions 46
— structure of Riemann surface for
Bloch functions S3
— theorem on approximation 63
— theorem on completeness for products
of Bloch functions 66
Spectral theory of two-dimensional
periodic Schrudinger operators
— 'finite zone with respect to level Eo'
two-dimensional periodic Schrudinger
operators 95
— perturbation theory for formal Bloch
solutions 85
— structure of complex 'Fermi
curves' 90
103