Solutions to I.E. Irodov’s Problems in General Physics Volume I
Mechanics • Heat • Electrodynamics
SECOND EDITION
ABHAY KUMAR SINGH
Director Abhay’s I.LT. Physics Teaching Centre Patna-6
CBS PUBLISHERS & DISTRIBUTORS
4596/1A, 11 DARYAGANJ, NEW DELHI -110 002 (INDIA)
Dedicated to my Teacher Prof. (Dr.) J. Thakur (Department of Physics, Patna University, Patna-4)
ISBN: 81-239-0399-5
First Edition: 1995
Reprint: 1997
Second Edition : 1998
Reprint: 2000
Reprint: 2001
Reprint: 2002
Reprint: 2003
Reprint: 2004
Reprint: 2005
Copyright © Author & Publisher
All rights reserved. No part of this book may. be reproduced or transmitted in any form or by any means, electronic or mechanical, including photocopying, recording, or any information storage arid retrieval system without permission, in writing, from the publisher.
Published by S.K. Jain for CBS Publishers & Distributors, 4596/1 A, 11 Darya Ganj, New Delhi - 110 002 (India)
Printed at :
India Binding House, Delhi - 110 032
FOREWORD
Science, in general, and physics, in particular, have evolved out of man’s quest to know beyond unknowns. Matter, radiation and their mutual interactions are basically studied in physics. Essentially, this is an experimental science. By observing appropriate phenomena in nature one arrives at a set of rules which goes to establish some basic fundamental concepts. Entire physics rests on them. Mere knowledge of them is however not enough. Ability to apply them to real day-to-day problems is required. Prof. Irodov’s book contains one such set of numerical exercises spread over a wide spectrum of physical disciplines. Some of the problems of the book long appeared to be notorious to pose serious challenges to students as well as to their teachers. This book by Prof. Singh on the solutions of problems of Irodov’s book, at the outset, seems to remove the sense of awe which at one time prevailed. Traditionally a difficult exercise to solve continues to draw the attention of concerned persons over a sufficiently long time. Once a logical solution for it becomes available, the difficulties associated with its solutions are forgotten very soon. This statement is not only valid for the solutions of simple physical problems but also to various physical phenomena.
Nevertheless, Prof. Singh’s attempt to write a book of this magnitude deserves an all out praise. His ways of solving problems are elegant, straight forward, simple and direct. By writing this book he has definitely contributed to the cause of physics education. A word of advice to its users is however necessary. The solution to a particular problem as given in this book is never to be consulted unless an all out effort in solving it independently has been already made. Only by such judicious uses of this book one would be able to reap better benefits out of it.
As a teacher who has taught physics and who has been in touch with physics curricula at I.I.T., Delhi for over thirty years, I earnestly feel that this book will certainly be of benefit to younger students in their formative years.
Dr. Dilip Kumar Roy Professor of Physics Indian Institute of Technology, Delhi New Delhi-110016.
FOREWORD
A- proper understanding of the physical laws and principles that govern nature require solutions of related problems which exemplify the principle in question and leads to a better grasp of the principles involved. It is only through experiments or through solutions of multifarious problem-oriented questions can a student master the intricacies and fall outs of a physical law. According to Ira M. Freeman, professor of physics of the state university of new Jersy at Rutgers and author of 4'physic—principles and Insights” — "In certain situations mathematical formulation actually promotes intuitive understanding....Sometimes a mathematical formulation is not feasible, so that ordinary language
must take the place of mathematics in both roles. However, Mathematics is far more rigorous and its concepts more precise than those of language. Any science that is able to make extensive use of mathematical symbolism and procedures is justly called an exact science”. I.E. Irodov’s problems in General Physics fulfills such a need. This book originally published in Russia contains about 1900 problems on mechanics, thermodynamics, molecular physics, electrodynamics, waves and oscillations, optics, atomic and nuclear physics. The book Jias survived the test of class room for many years as is evident from its number of reprint editions, which have appeared since the first English edition of 1981, including an Indian Edition at affordable price for Indian students.
Abhay Kumar Singh’s present book containing solutions to Dr. I.E. Irodov’s Problems in General Physics is a welcome attempt to develop a student’s problem solving skills. The book should be very useful for the students studying a general course in physics and also in developing their skills to answer questions normally encountered in national level entrance examinations conducted each year by various bodies for admissions to professional colleges in science and technology.
B.P. PAL Professor of Physics I.I.T., Delhi
PREFACE TO THE SECOND EDITION
Nothing succeeds like success, they say. Now, consequent upon the warm welcome on the part of students and the teaching fraternity this revised and enlarged edition of this volume is before you. In order to make it more up-to-date and viable, a large number of problems have been streamlined with special focus on the complicated and ticklish ones, to cater to the needs of the aspiring students.
I extend my deep sense of gratitude to all those who have directly or indirectly engineered the cause of its existing status in the book world.
Patna
June 1997
Abhay Kumar Singh
PREFACE TO THE FIRST EDITION
When you invisage to write a book of solutions to problems, one pertinent question crops up in the mind that—why solution! Is this to prove one’s erudition? My only defence against this is that the solution is a challenge to save the scientific man hours by channelizing thoughts in a right direction.
The book entitled “Problems in General Physics” authored by I.E. Irodov (a noted Russian physicist and mathematician) contains 1877 intriguing problems divided into six chapters.
After the acceptance of my first book “Problems in Physics”, published by Wiley Eastern Limited, I have got the courage to acknowledge the fact that good and honest ultimately win in the market place. This stimulation provided me insight to come up with mv second attempt—“Solutions to I.E. Irodov’s Problems in General Physics.”
This first volume encompasses solutions of first three chapters containing 1052 problems. Although a large number of problems can be solved by different methods, I have adopted standard methods and in many of the problems with helping hints for other methods.
In the solutions of chapter three, the emf of a cell is represented by § (xi) in contrast to the notation used in figures and in the problem book, due to some printing difficulty.
I am thankful to my students Mr. Omprakash, Miss Neera and Miss Punam for their valuable co-operation even In my hard days while authoring the present book. I am also thankful to my younger sister Prof. Ranju Singh, my younger brother Mr. Ratan Kumar Singh, my junior friend Miss Anupama Bharti, other well wishers and friends for their emotional support. At last and above all I am grateful to my Ma and Pappaji for their blessings and encouragement.
ABHAY KUMAR SINGH
CONTENTS
Foreword	iii
Preface to the second edition	v
Preface to the first edition	vi
PART ONE PHYSICAL FUNDAMENTALS OF MECHANICS
1.1	Kinematics	1-34
1.2	The Fundamental Equation of Dynamics	35-65
1.3	Laws of Conservation of Energy, Momemtum, and Angular Momentum 66-101
1.4	Universal Gravitation	102-117
1.5	Dynamics of a Solid Body	118-143
1.6	Elastic Deformations of a Solid Body	144-155
1.7	Hydrodynamics	156-167
1.8	Relativistic Mechanics	168-183
PART TWO THERMODYNAMICS AND MOLECULAR PHYSICS
2.1	Equation of the Gas State. Processes	184-195
2.2	The first Law of Thermodynamics. Heat Capacity	196-212
2.3	Kinetic theory of Gases. Boltzmann’s Law and Maxwell’s Distribution 213-226
2.4	The Second Law of Thermodynamics. Entropy	227-241
2.5	Liquids. Capillary Effects	242-247
2.6	Phase Transformations	248-256
2.7	Transport Phenomena	257-266
PART THREE ELECTRODYNAMICS
3.1	Constant Electric Field in Vacuum	267-288
3.2	Conductors and Dielectrics in an Electric Held	289-305
3.3	Electric Capacitance. Energy of an Electric Held	306-324
3.4	Electric Current	325-353
3.5	Constant Magnetic Held. Magnetics	354-379
3.6	Electromagnetic Induction. Maxwell’s Equations	380-407
3.7	Motion of Charged Particles in Electric and Magnetic Helds	408-424
PART ONE
PHYSICAL FUNDAMENTALS OF MECHANICS
1.1 KINEMATICS
1.1 Let be the stream velocity and v' the velocity of motorboat with respect to water. The motorboat reached point В while going downstream with velocity (vQ + v') and then returned with velocity (v' - and passed the raft at point C. Let t be the time for the raft (which flows with stream with velocity	to move from point A to C, during which the motorboat
moves from A to В and then from В to C. Therefore
*---------»
v0	(v-Vo)	Д.----------------------------
On solving we get vQ« —	** C
1.2 Let s be the total distance traversed by the point and rx the time taken to cover half the distance. Further let 2t be the time to cover the rest half of the distance.
Therefore	y- vorx or zi“	(!)
and	(vj + vJr or 2r- —-—	(2)
2	1 v	Vj + v2
Hence the sought average velocity
_ S _________________S____________ 2vo(vl + v2)
<V>" ti + 2t~ [s/2vo]+[s/(v1 + v2)]~ v1 + v2 + 2v0
13 As the car starts from rest and finally comes to a stop, and the rate of acceleration and deceleration are equal, the distances as well as the times taken are same in these phases of motion.
Let Ar be the time for which the car moves uniformly. Then the acceleration / deceleration
time is
т - Ar , o —-— each. So,
or
Hence
Дг« т
= 15 s.
1.4 (a) Sought average velocity
200 cm
20s
10 cm/s
^y
(b) For the maximum velocity, — should be
maximum. From the figure — is maximum for all points on the line ac, thus the sought maximum velocity becomes average velocity for the line ac and is equal to :
be ab
100 cm 4s
«25 cm/s
5 <v> « t
(c) Time should be such that corresponding to it the slope — should pass through the
point О (origin), to satisfy the relationship • —. From figure the tangent at point d at t0
passes through the origin and thus corresponding time t« tQ « 16 s.
13 .Let the particles collide at the point A (Fig.), whose position vector is (say). If t be the
time taken by each particle to reach at point A, from triangle law of vector addition :
1.6 Wehave
V - V-Vo
(1)
From the vector diagram [of Eq. (1)] and using properties of triangle
3
and
or
Vq + v2 + 2 v0 v cos ф « 39.7 km/hr (2) v'	v	. n	v sin qp
-----------=		or,	sin	0	=  L-sm (л - ф) sin 0	v
n	.	sin ф\
0*	sin	—,
I	V	I
Using (2) and putting the values of v and d 0 « 19.1*
1.7 Let one of the swimmer (say 1) cross the river along AB, which is obviously the shortest path. Time taken to cro& the river by the swimmer 1.
, (where AB « d is the width of the river)	(1)
12	2
V v' - Vo
For the other swimmer (say 2), which follows the quickest path, the time taken to cross the river.
In the time r2, drifting of the swimmer 2, becomes
x « Vo t2 « у d, (using Eq. 2)	(3)
If t3 be the time for swimmer 2 to walk the distance x to come from C to В (Fig.), then у Vn d	,
(4)
According to the problem « t2 +13 d d	vod
or,	—== « “7 + “7—
V ,2 2 V	Vu
YV -Vo
On solving we get и -------------------------------------- з km/hr.
4
1Л Let / be the distance covered by the boat A along the river as well as by the boat В acre the river. Let v0 be the stream velocity and v' the velocity of each boat with respect water. Therefore time taken by the boat A in its journey
I I
*л " V + v0 + V - v0
and for the boat В tB-	£--,£ +	2 j 
V v - v0 V v - v0 V v ~ v0
„	{A v' _________________n ( , v' \
Hence,	— =» / „ 	» ~v~-w where *n = — I
On substitution tA/tB «1-8
1Э Let v0 be the stream velocity and v' the velocity of boat with respect to water. A v0
~ - T) - 2 > 0, some drifting of boat is inevitable.
Let v* make an angle 0 with flow direction. (Fig.), then the time taken to cross the rive
t« ;	 (where d is the width of the river)
v sin 0	'
In this time interval, the drifting of the boat x« (v'cos0 + vo)t
* (v' cos 0 + Vq) « (cot 0 + T) cosec 0) d
For x^ (minimum drifting)
777 (cot 0 + T] cosec 0)« 0, which yields d 0
COS0- - —- -i-
T) 2
Hence,	0- 120°
1.10 The solution of this problem becomes simple in the frame attached with one of the bodies. Let the body thrown straight up be 1 and the other body be 2, then for the body 1 in the frame of 2 from the kinematic equation for constant acceleration :
—► —>	—>	1 —> 2
r12“ r0(12) + v0(12)* + 2M'12r
So, ^12" ^12)(because 0 and °)
OI, 1^1- 1^0(12)1*	(1)
So,	from properties of triangle
Vo(12)= V Vq +- 2 v0 v0 cos (jt/2 - 0O)
Hence, the sought distance
I ''is I * vo V 2 (1 - sin 0) t - 22 m.
5
Let the velocities of the parities (say time t. Then their velocitis become
——♦ —> —>' —> —* vi “ vx + gt; v2 - v2 + gt
As \\ ± so,	« 0
or, (^+Fr) (^'+Fr)"’o or	-v1v2 + Fr2“ 0
тт	V v,v2
Hence, t«  g
and ) becomes mutually perpendicular after
—>	—-J*	—I  > 2
Now form the Eq. r12 «	+ v(X12) t + ~ w121
I г121 “ I VO(12) I (because here w12 • 0 and - 0)
Hence the sought distance
I ^12 I " ~i~V'vlv2 (as I ^0(12) I “ V1 + v2> О
1.12 From the symmetry of the problem all the three points are always located at the vertices of equilateral triangles of varying side length and finally meet at the centriod of the initial equilateral triangle whose side length is a, in the sought time interval (say t).
Let us consider an arbitrary equilateral triangle of edge length I (say).
Then the rate by which 1 approaches 2, 2 approches 3, and 3 approches 1, becomes :
-dl	(2я\
—- Ж V - V COS —T—
Jr	I 3 I
On integrating :
о	t
/л 3v Сл dl = — J dt
a	0
3 a « — vf so
2a
3v
6
1.13
Let us locate the points A and В at an arbitrary instant of time (Fig.).
If A and В are separated by the distance 5 at this moment, then the points converge or — ds
point A approaches В with velocity « v - и cos a where angle a varies with time.
On inteigating, о	т
J*(v - и cos a) dt, i	о
(where T is the sought time.) т
or I- J\v-и cos a)dt о
As both A and В cover the same distance in x-direction during the sought time interval, so the other condition which is required, can be obtained by the equation
Ar-fvxdt
T
So,	uT- J*v cos a dt	(2)
о
Solving (1) and (2), we get T« a—-a
ir-u
One can see that if и « v, or и < v, point A cannot catch B.
1.14 In the reference frame fixed to the train, the distance between the two events is obviously equal to L Suppose the train starts moving at time t« 0 in the positive x direction and take the origin (x « 0 ) at the head-light of the train at t« 0. Then the coordinate of first event in the earth’s frame is
and similarly the coordinate of the second event is
X2-+
The distance between the two events is obviously.
x1-x2« / - wt (t + t/2) «0-242 km
in the reference frame fixed on the earth..
For the two events to occur at the same point in the reference frame X, moving with constant velocity V relative to the earth, the distance travelled by the frame in the time interval T must be equal to the above distance.
Thus	Vx «I - wx( t + t/2 )
So,	v«l_w(f + T/2) -4-03 m/s
The frame К must clearly be moving in a direction opposite to the train so that if (for example) the origin of the frame coincides with the point xx on the earth at time t, it coincides with the point x2 at time t + t.
7
1.15 (a) One good way to solve the problem is to work in the elevator’s frame having the observer at its bottom (Fig.).
Let us denote the separation between floor and celing by h - 2*7 m. and the acceleration of the elevator by w « 1*2 m/s2
From the kinematical formula
1	2
у-Уо + М+2 v W
Here у - 0, y0 - + h, - 0 and wy-
- (-g)-(w)- -(g + w)
So, 0» +	+
or, t =•	- « 0-7 s.
' g + w
(b) At the moment the bolt loses contact with the elevator, it has already aquired the
velocity equal to elevator^ given by :
v0 - (1*2) (2)- 2*4 m/s
In the reference frame attached with the elevator shaft (ground) and pointing the у-axis upward, we have for the displacement of the Ьо1Ц 1	2
Ay- v^t + ^Wyt
- v0t+j(-g)t2
or,	Ду - (2-4) (0-7) +1 (-9-8) (0-7)2- -0-7m.
Hence the bolt comes down or displaces downward relative to the point, when it loses contact with the elevator by the amount 0*7 m (Fig.).
Obviously the total distance covered by the bolt during its free fall time (2\	2
^1 = 0-7m + ^j-m- 1-3 m.
1.16 Let the particle 1 and 2 be at points В and A at t« 0 at the distances and Z2 from intersection point O.
Let us fix the inertial frame with the particle 2. Now the particle 1 moves in relative to this reference frame with a relative velocity v^2 « and its trajectory is the straight line BP. Obviously, the minimum distance between the particles is equal to the length of the perpendicular AP dropped from point A on to the straight line BP (Fig.).
8
The shortest distance
AP « AM sin 0 « (QA - OM) sin 0 « (/2 ~ cot 0) sin 0
(v9\ V! v, L - v9 L
h. ” h	j'T—7 * -/ 2~ ”2 ‘ (using 1)
viy V vx + Vq, * vi+ V2
The sought time can be obtained directly from the condition that (lr - vx r)2 + (/2 - v2 r)2
/x vx +12 v2
is minimum. This gives t « —»-*— .
V1 + V2
1.17 Let the car turn off the highway at a distance x from the point D,
So, CD « x, and if the speed of the car in the field is v, then the time taken by the car to cover the distance AC « AD -x on the highway
AD-x t ж ----
1 T| v
and the time taken to travel the distance CB in the field
So, the total time elapsed to move the car from point A to В
t-
AD-x >/ l2 + x? ------+------
T] V	V
For t to be minimum
or
Г|2Х2« /2+Х2 ОГ X»  ; ;
9
1.18 To plot x (0, s (0 and wx (t) let us partion the given plot vx (r) into five segments (for detailed analysis) as shown in the figure.
For the part oa : wx « 1 and vx « t« v
Г	t2
y- S1(t) о
Putting Г» 1, we get, Axj« unit

For the part ab : wr — О and
v « v « constant« 1
11 n< li
1 О
е
Thus Putting	^2 W “ J “ J dt “ (* " 1) “ s2 W 1 3, Лх2« s2« 2 unit
For the part M	: wx « 1 and vx « 1 - (t - 3) « 4 -t) « v
Thus	f	t2 15 AM')-J(4-r)t*- 4r-^-y- s3(t) 3
Putting	t- 4, A x, « x, « 77 unit 2
For the part 4J	:	vx = - 1 and vx = - (1 - 4) = 4-1
So,	v = | vx | - t - 4 for t > 4
Thus	t Ax4(t)-4r-y-8 4
Putting	t = 6, A x4 = - 1 t
Similarly	2 s4(0 = Jl vx\dt^ f(t-4)dt» y-4t-4
Putting	6, s4« 2 unit
For the part d 7	:	wx = 2 and vx « - 2 + 2 (t - 6) « 2(t v- | vx | « 2(7-0 for r<-7 6
Now,	Дх(г)-J2 (t-7)dt~ r2-14t + 48
Putting	t = 4, Дх5 « - 1 6
Similarly	s5(0-f2(7-t)dt~ 14r-Z2-48
Z = 7, 55 « 1
Putting
-7)
On the basis of these obtained expressions (r), x (r) and 5 (/) plots can be easily plotted
as shown in the figure of answersheet.
10
1.19 (a) Mean velocity
Total distance covered <v> _ -----—-----------------
Time elapsed
«	50cm/s (1) Vq
t %	v 7
(b)	Modulus of mean velocity vector ,	. I Ari 2R __	,
< v > « J——L« ------ж 32 cm/s (2)
Ar x
(c)	Let the point moves from i to /along the half circle (Fig.) and v0 and v be the spe at the points respectively.
We have	- wt
dt 1
or, v = v0 + wt t (as wt is constant, according to the problem) t f (v0 + w,t)dt
_	о	v0 + (v0 + w/) v0+v
So,	<v> - ------------« -------------- —-—
‘	2	2
p 0
So, from (1) and (3) Vp + V hR 2 ~ x
Now the modulus of the mean vector of total acceleration
c
(3
(5
Using (4) in (5), we get :
1.20 (a) we have	r « a t (1 - a r)
So,	v^= a*(l - 2 a f)
’	dt v 7
.	-* dv -*
and	w -2aa
at
(b) From the equation Г» ant (1 - a t),
r^ 0, at t = 0 and also at t - ^t= ~ a
So, the sought time Ar = ~
As	v^= a*(l - 2 a t)
So,
a(l-2at)l forrs v=|v[=	j
a (2 a t - 1) for t > -—
J 2 a
11
Непсе, the sought distance 1/2 a	1/a
s=J*vdr=Jt a(l-2ar)dr + J* a(2af-l)^ 0	1/2 a
Simplifying, we get, 5 = ~~~
1.21 (a) As the particle leaves the origin at t = 0
So,	Ax « x « J*vx Jr	(1)
As	v^= v^| 1 - -|,
\ V where v0 is directed towards the +ve x-axis
So>	(2)
From (1) and (2), t
X"	Vo'(1_2t)	(3)
0	'	'	'	'
Hence x coordinate of the particle at t = 6 s.
x = 10 x 6 11 - J « 24 cm = 0-24 m
I 2x51
Similarly at	r = 10 s
x = 10 X 10 fl -	= 0
I 2x5l
and at	r - 20 s
(20 \ l-^r« -200 cm = -2m 2x5y
(b) At the moments the particle is at a distance of 10 cm from the origin, x = ± 10 cm. Putting	x = + 10 in Eq. (3)
10= 10^1-^) or, r2-10r + 10= 0,
o	10 ± V100 - 40	.
So,	r « r » -------------= 5 ± v 15 s
Now putting	x = - 10in Eqn (3)
-10-10(1-s)'
On solving,	t - 5 ± >/35 s
As r cannot be negative, so,
t-(5+V35 )s
12
Непсе the particle is at a distance of 10 cm from the origin at three moments of time :
s
(c) We have
V- VO
So,
V-H- V”Г’(
for/s т
for t > T
So
Jv011 - -| di for t * % » v01 (1 - #r) о \	/
and
dt for t>x
о	' т
- v0t[1+(1-54)2]/2 for г>т
4	4
(A)
J V° о
dt = 24 cm.
And for t = 8 s
5
s - J 10 11 - Ij Л +J 1° (J - 1 j dt o''	5	'	'
On integrating and simplifying, we get
5 « 34 cm.
On the basis of Eqs. (3) and (4), x (r) and s (t) plots can be drawn as shown in the answer sheet
1.22 As particle is in unidirectional motion it is directed along the x-axis all the time. As at t = 0, x = 0
So,
A
Ar = x = s, and — « w ’ dt
Therefore,
or,
dv w « — dt
q ds a 2 Vs dt	2 Vs
q2
As,
q v q q V2
“ 2Vs 2 Vs
dv a2 и»- —- —
dt 2
(1)
On integrating,
, j a2 , dv = I — di or, J 2
(2)
s «
о
8
о
2
о
13
(b) Let 5 be the time to cover first s m of the path. From the Eq.
5 = J* vdt t /2	2/2
CL . U t	7 •
~2dt°~2^2	(using 2)
0
2 Г or	r= — yjs
a
The mean velocity of particle
(3)
2*/у/а
1.23 According to the problem
—  aVv (as v decreases with time) ds	4
or,
0
2 3/2
On integrating we get s » — v0
Again according to the problem
dv г dv .
aNv or t=--= adt dt	Vv
or, Thus
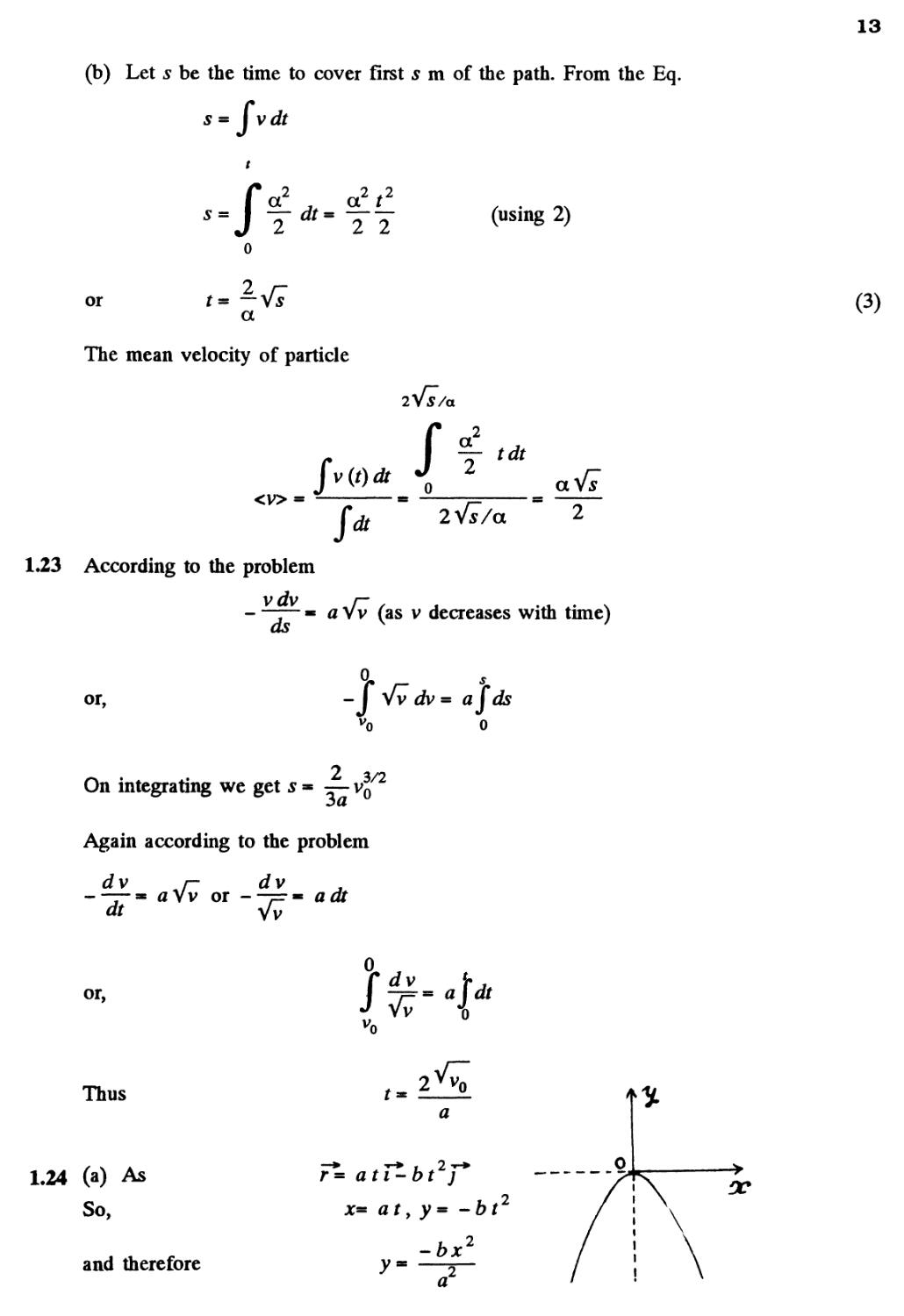
1.24 (a) As So, and therefore
a I* dt о
vo
14
which is Eq. of a parabola, whose graph is shown in the Fig.
(b)	As	ati^bt2r
dr* -г* „,	-«-*	z_4
v - ai-2btj	(1)
So,	v = Va 2 (- 2 b t )2 = V a2 + 4b2tz
Diff. Eq. (1) w.r.t. time, we get -*	-I.’*
w-—--26j
So,	| v?| = w = 2b
..	(ai^-2btj*)-(-2bj]
(c)	cos a - ------------»	______
vw pa2 + 4b2t2)2b
2bt or,	cos a =	===,
V a2 + 4b2t2
a
so,	tan a = —:—
’	2bt
or, a = tan
(d)	The mean velocity vector
< v > =
ai^-btf*
Hence,
| < v* > I - Va2+ (-bt)z - ^a'l + b2t2
1.25 (a) We have
x = a t and y- at(l-at)
(1)
Hence, у (x ) becomes,
ax L ax a 2 (	, 4
1 - I = x - — x (parabola)
(b) Diiferentiating Eq. (1) we get
vx = a and vy = a (1 - 2 a t)	(2)
15
So,	v « Vvx + Vy = a Vl + ( 1 -2ar)2
Diff. Eq. (2) with respect to time
wx= 0 and wy = -2aa
So,	w « V w? + w 2 = 2 a a
* У
(c) From Eqs. (2) and (3)
We have	a Y+ a ( 1 - 2 a t )J* and 2 adj"*
o	я 1	-a(l-2af0)2aa
So,	cos - = -7= =-= —........ ..	----
4 V2 vw aV1 + (1-2af0)2 2лa
On simplifying.
1 - 2 a tQ - ±1
As,	*o#O, *oe a
1.26 Differentiating motion law : x « a sin cd t, у s a (1 - cos cd r), with respect to time, v = a cd cos cd?, v = a cd sin cd*
x	У
So,	v^= a cd cos cd*/+ a co sin co* J*	(1)
and	v = a co « Const	(2)
Differentiating Eq. (1) with respect to time
-* с/ v	2 .	_ t* 2	z^\
w = — = - a cd sin art 1 + a cd cos ortj	(3)
at
(a)	The distance 5 traversed by the point during the time % is given by
s « J* v dtж Ja wdt- а а) т (using 2) о 0
(b)	Taking inner product of v* and w
We get,	(a cd cos cor i + a co sin artj )• (a co2 sin mt ( - i) + a co2 cos cor - j )
So,	w = - a2 cd2 sin co* cos co* + a2 co3 sin cd* cos cd* = 0
Thus, vtL w, i.e., the angle between velocity vector and acceleration vector equals у.
1.27 Accordiing to the problem
w= w(-j)
So,
dv.	dv
w, = —r- “ 0 and w - -r2-» -w * dt	у dt
(1)
(2)
2	•
Differentiating Eq. of trajectory, у « ax - bx , with respect to time
dt
adx dt
-2bx
dx dt
16
So,
dy dt
x-0
dx = a — dt
Again differentiating with respect to time d2y ad2x ^.(dx\2 d2x
—T=  y-“2b — -2b x—г dt2 dt2 dt I	dt2
or,
/	, x2
-w= a (0) - 2 b I ~~~~~~ I - 2 bx (0) (using 1)
or,
dx	z .
~л'Угь (“smgl)
(-
Using (3) in (2)
djL dt
x- 0
Hence, the velocity of the particle at the origin
(using Eqns (3) and (4))
Hence,
v =
1.28 As the body is under gravity of constant accelration g , it’s velocity vector and displacemen vectors are:
+ F	(i.
and	Ar = r - v01 + — g*2 (r = 0 at t - 0)	(2}
So,	<v> over the first t seconds
(4)
-> Ar r -> gt
<V> = — = ~=vn + ^	(3)
Ar t 0 2	v 7
Hence from Eq. (3), <v> over the first t seconds
<V>~ K+2
For evaluating t, take
(F+FHF+FO- Vo + 2(vF' + g2'2
or, v2= Vo+(y£g}t + g2t2
But we have v = v0 at t = 0 and
Also at t = т (Fig.) (also from energy conservation)
17
Hence using this propety in Eq. (5)
Vo- Vo + 2(v^gTT + gV
As
SO, T=----------5--
g
Putting this value of т in Eq. (4), the average velocity over the time of flight
<V>= vo-g--2
g
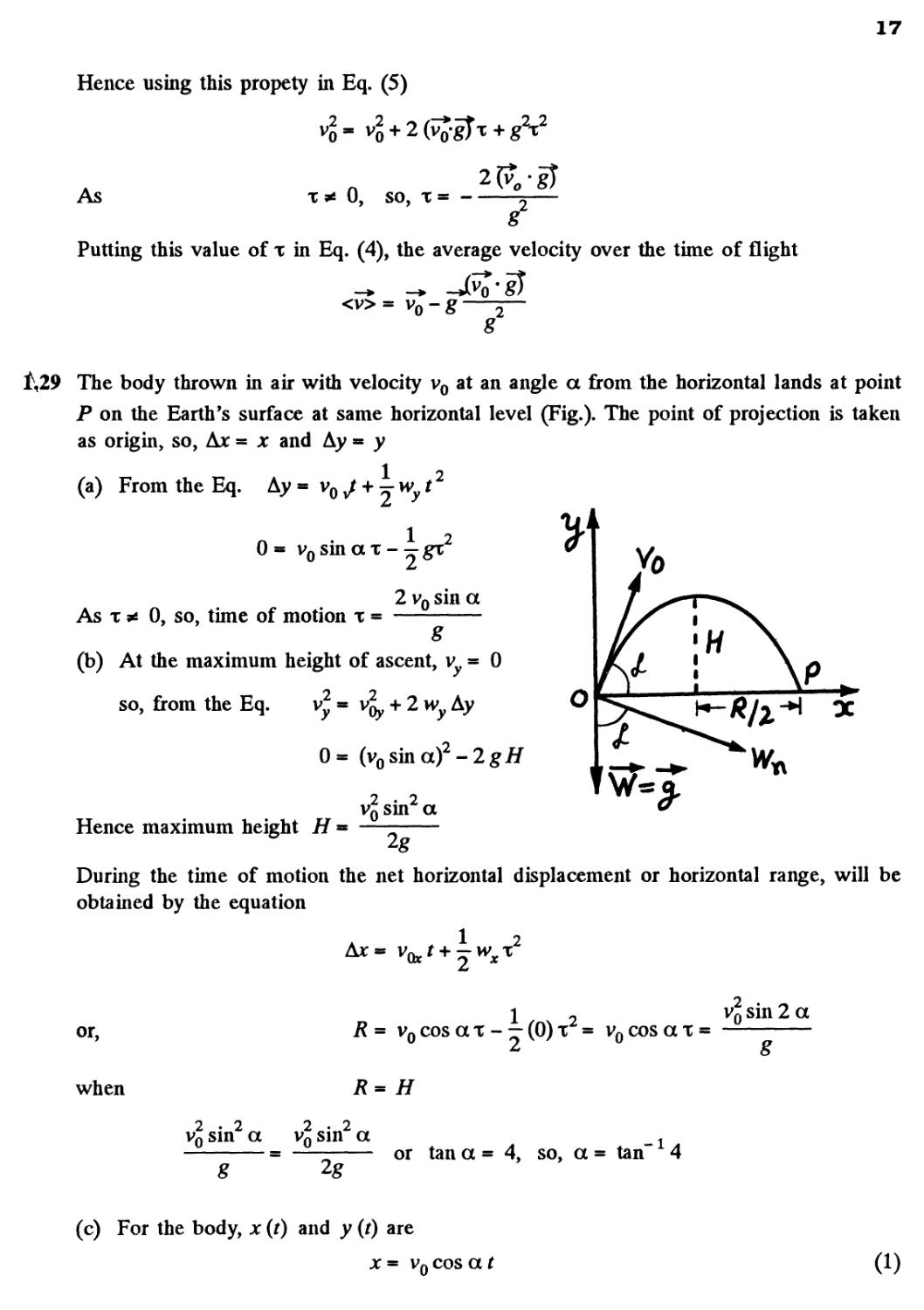
1,29 The body thrown in air with velocity v0 at an angle a from the horizontal lands at point P on the Earth’s surface at same horizontal level (Fig.). The point of projection is taken as origin, so, Ax = x and Ay = у
1	2
(a)	From the Eq. Ay = v0 / + J wy1
0 = v0 sin а т - gx2
2 v0 sin a As т * 0, so, time of motion т = ---
g
(b)	At the maximum height of ascent, vy = 0
so, from the Eq. v2 =	+ 2 wy Ay
0= (v0 sin a)2 - 2 g#
2 • 2 vosm a Hence maximum height H = —-------
During the time of motion the net horizontal displacement or horizontal range, will be obtained by the equation
1	2
2 • ~
1	2	v0 sin 2 a
or,	R = v0 cos a x “ 2 (0)T ~ v° cos a T = -~---
when	R - H
2«2	2*2
vosin a vosin a	t
	= —--- or tan a = 4, so, a = tan” 4 g------------------2g
(c) For the body, x (r) and у (r) are
x = v0 cos a t
(1)
18
and
•	1	2
У = vosmar--gr
(2)
Hence putting the value of t from (1) into (2) we get, /	\	1	/	\2	2
( X \	1 ( X \ A	gx
y — v0since ------- -~g --------- «xtana-—f—z—,
^cosaj 2 ^v0cosaj	2vocos2a
Which is the sought equation of trajectory i.e. у (x)
(d) As the body thrown in air follows a curve, it has some normal acceleration at all the
moments of time during it’s motion in air.
At the initial point (x = 0, у = 0), from the equation :
v2
~, (where R is the radius of curvature)
v0 z	_ v „	vo
8 cos a « F- <see F«g ) or Ro -AVQ	g Wo U<
At the peak point vy = 0, v = vx = v0 cos a and the angential acceleration is zero.
v2
Now from the Eq.	~
2	2	2	2
v0 cos a	v0 cos a
s-—j—, » K-——
Note : We may use the formula of curvature radius of a trajectory у (х\ to solve part (d),
Г	2 15
*'	l^y/Л2!
1.30 We have, vx = v0 cos a, vy = v0 sin a - gt As	f ut all the moments of time.
Thus
Now,
Hence
v2 == vt2 - 2 gt v0 sin a + g212 dv< 1 d / x 1	.	- x
о
- -5.(vosina-gt)- -g-r-vt	vt
I I I vy I
I wt I = g—f—
Now wn = V -wf -V g‘
or
v ,
“ g— (where n vt \
19
As	t vt, during time of motion
v "v =	= “g
On the basis of obtained expressions or facts the sought plots can be drawn as shown in the figure of answer sheet
1.31 The ball strikes the inclined plane (Ox) at point О (origin) with velocity v0 = V 2gh (1) As the ball elastically rebounds, it recalls with same velocity v0, at the same angle a from the normal or у axis (Fig.). Let the ball strikes the incline second time at P, which is at a distance I (say) from the point Ot along the incline. From the equation
1	2
yss v0yt + 2Wyf
where т is the time of motion of ball in air while moving from О to P.
2v0
As 0, so, t= -------- (2)
g
Now from the equation.
1	2
* = v(kf + 2 Wxt
j .	1.2
I = v0 sin а т + — g sm а т
4 v? sin a
----------- (using 2)
О
тт	vx ,!- . i 4 (2 gh) sin a o, . /TT .	„
Hence the sought distance, I- s °- 7-------= 8Л sm a (Using Eq. 1)
g
1.32 Total time of motion
2v0sina . xg 9-8t g	2v0
and horizontal range n	R
R= vft cos at or cos a - -------=
°	V0T
(1)
(2)
5100	85
240т ° 4t
From Eqs. (1) and (2)
(9-8)2t2	(85)2 _
(480)2	(4 т2)2
On simplifying
t4 — 2400 t2 + 1083750 = 0
20
1.33
1.34
Solving for t2 we get :
2400 ± V 1425000	2400 ± 1194
2	2
42-39 s = 0-71 min and
Thus
,2
24-55 s « 0-41 min depending on the angle a.
Let the shells collide at the point P (x, y). If the first shell takes t s to collide With second and Ar be the time interval between the firings, then
x = v0 cos 0! t = v0 cos 02 (t - A r) (1) 1	2
and y = vosin01r--gt
= vosin02(t-At)-jg(t-At)2 (2)
Ar cos 0?
From Eq. (1) r = -----------—
ч v 7 cos 02 _ cos 01
From Eqs. (2) and (3)
2 v0 sin (et - e2)
g (cos 02 + COS 0!) According to the problem
(a) Vo or v^dt
(3)
as Ar* 0
О
Vf
V-2

Integrating
And also we have
о dx ~dt’ay
V° J о
V
(1)
or
dx =
aydt» avQtdt (using 1)
So,
I tdt, о
or,
(b)
о According to the problem
vy « v0 and vx = a у
1	2 1 ay2 . .
2ЙУ°Г = 2-V<USmgl)
(2)
So,
Therefore
dv dt
2	2	\/ 2	2 2
vJ + v>,“Vv0 + ay
2 j	2
a y dy a у
'l + ay2 dt V 1 + /fly
Diff. Eq. (2) with respect to time.
d v
= w = 0 and dt у
dvx	dy
—— = w = a-j- = avQ dt x dt 0
So,
w = I wx I = a v0
21
Hence n
2
1.35 (a) The velocity vector of the particle a i + bx j
V»
So,
dx dt “
a : ~r~ = bx dt
From (1)
о
a J dt or, x = at о
And
dy
bxdt= batdt
Integrating
о
о
1,2 2abt
From Eqs. (2) and (3), we get, у
(b) The curvature radius of trajectory у (x) is : з
[l + Gfy/d’O2 ]3
—x2 with respect to x,
d2 у b
and —==•= —
dx2 «
From Eqs. (5) and (6), the sought curvature radius : з 2
Let us differentiate the path Eq. у
£
dx a
(1)
(2)
(3)
(4)
(5)
(6)
b \2
—xl a
1.36 In accordance with the problem
But

So,
a -T vdv ds or (a*-T^ds= a*-dr
vdv = wt ds
or,
vdv
vdv= a i- dr = adx (because a is directed towards the x-axis)
V	X
So,
J vdv = a J dx о	0
Hence
Q		I.. I
v = 2 ax or, v “ v2 ax
22
1.37
The velocity of the partide v - at
So,
And
dv
— = w- a dt '
vI 2 * * a2t2 ( .
I' T" (usm8v“ar>
(1)
(2)
From
/1 о vdt» 2at
о
So,
4 лт] t2 a ~ R
(3)
From Eqs. (2) and (3) wn « 4лат)
Hence w= V
« у/ a2 + (4o т])2 « a V 1 + 16 t)2 « 0-8 m/s2
1.38 According to the problem
For v (f),
1^1- |wj
-dv v2 dt " R
Integrating this equation from v0 £ v £ v and 0 £ t * t
I dv 1 I	v0
“I ~7 s 7 I ОГ, V = --------------г
J RJ 1 + M-
V. °	I1+ Я I
2	'	7
V dv V
Njw for v (s)y -	~ , Integrating this equation from v0 s v s v and 0 s js s
Hence	v« voe”
(b) The normal acceleration of the point v2 v2e-^ И'"ж Я = —Я--------------------------- (Using
And as accordance with the problem
|wj- | Wn | and w,utLwnun
(2)
so,
v2	2
w= V2wrt=^^e-^= V2
23
1.39 From the equation
dv	a ds a
gg mm_ 2	———— —— 2jj ж——
' dt	2Vs dt 2Vs
2
w » — « ——
* R R
As w, is a positive constant, the speed of the partide increases with time, and the tangential acceleration vector and velodty vector coincides in direction.
_A	_»
Hence the angle between v and w is equal to between wti»t an w, and a can be found
. t л * i	I I a2s/R 2s
by means of the formula : tan a »—r « —z-» —
HI a2/2 Л
1.40 From the equation I« a sin cd t
dl
— « у» a co cos со г dt
-	2	.	J	/4 \
So, — - -a cd sin cor, and	(1)
v2 a2 cd2 cos2 co t	z 4
w « — « ---------------- (2)
* R R	v 7
(a) At the point I« 0, sin co r = 0 and cos cd t« ± 1 so, cor = 0, л etc.
„	a2 co2
Hence	w «—-—
* R
Similarly at I = ± a, sin cor« ± 1 and cos <nt « 0, so, wn « 0
Hence	w « | wj « a cd2
1.41 As wt« a and at t = 0, the point is at rest
So,	v(r) and s(t) are, v« at and ~ar2	(1)
Let R be the curvature radius, then
V2 a2t2 2as . .	..
И'"ш ~r-----------^_<USU1£ D
But according to the problem % -b(4
s°, dr4= or, Л= A; (using 1)	(2)
л\	£ OS
Therefore	w - V w2 +	= V a2 + (2 as/A)2 - V a2 + 4 bs2/a2)2(using 2)
Hence	w = a V 1 + f 4 bs2 / a3\2
24
1.42 (a) Let us differentiate twice the path equation у (x) with respect to time.
|2	d2x
I	dt2
dy	_ dx	try
2ax — \	~4--	2a
dt	dt	dt2
Since the particle moves uniformly, its acceleration at all points of the path is normal and at the point x » 0 it coincides with the direction of derivative d2y/dt2. Keeping in mind
that at the point x « 0,
dx dt
» v,
We get
dt2
- lav2» wt
X- 0
So,
(1)
2 y2	|
w  2 a v * —, or R - — n	R	2a
Note that we can also calculate it from the formula of problem (1.35 b) (b) Differentiating the equation of the trajectory with respect to time we see that , 2 dx 2 dy
which implies that the vector (b2xt*+ a2 у fl is normal to the velocity vector v 	F*+ which, of course, is along the tangent. Thus the former vactor is along
the normal and die normal component of acceleration is clearly
,2 <?X	2 <?"У
b x —=- + а у—t
dt2 dt2 wn~ / j.4 2 .	42 \l/2
on using wn =
0 , у = ± b and so at x = 0
Differentiating (1)
0 at x - 0 dt
Also from (1)
I  4 v (since tangential velocity is constant « v )
So
Thus
a2
and
fry2 a2
This gives R « aVb.

n
x- 0
2
0
R
25
1.43 Let us fix the co-ordinate system at the point О as shown in the figure, such that the radius vector r*of point A makes an angle 0 with x axis at the moment shown.
Note that the radius vector of the particle A rotates clockwise and we here take line ox as reference line, so in this case
R
0
angular velocity co»
anticlockwise sense of angular displacement as positive.
Also from the geometry of the triangle OAC
R	r	~ D Л
"T—- » T~7-----T-TT or, r - 2 Я COS 0.
sm 0 sm (л - 2 0) Let us write, Г» r cos 0	r sin 0 J== 2 R cos2 0R sin 2 0 j
Differentiating with respect to time.
—->
or v*» 2 R 2 cos 0 ( - sin 0)	/ + 2 R cos 2 0	7*
dt	dt 1	dt J

or, v*= 2R j [ sin 2 0 i - cos 2 Qj ]
or, Г= 2 R co (sin 2 0 i - cos2 0 j)
So,	I v| or v- 2co R -0-4 m/s,
d v
As co is constant, v is also constant and wt • — » 0, ’	‘dt
So,
w- wn-	4<о2Я- 0-32 m/s2
" R R
Alternate : From the Fig. the angular velocity of the point A, with respect to centre of the circle C becomes
<*(20) ,j-de\ „ t ,
-----*— « 2 —;— I« 2 co » constant dt I dt I
Thus we have the problem of finding the velocity and acceleration of a particle moving along a circle of radius R with constant angular velocity 2 co.
Hence	v= 2 co Я and
v2 (2ШЙ)2	2
w“ ~r“ -----------R-----4<0 R
1.44 Differentiating <p (t) with respect to time
-j*-» co = 2a t dt z
For fixed axis rotation, the speed of the point A:
2atR or R» ~— 2at
(1)
(2)
26
Differentiating with respect to time dv ~ D v , .	_
и',“ л" 2aR~ 7>(usmg
V2 v2
But	w - — » —“— » 2 a t v (using 2)
* R v/2at	\ ь J
So,	w - Vw2 + w2 « V (v/t)2 + (2atv)2
- —V”1 i4a2? t
1.45	The shell acquires a constant angular acceleration at the same time as it accelerates linearly. The two are related by (assuming both are constant)
w I
2nn
Where w - linear acceleration and 0 » jmgular acceleration
Then, co - V 2-р2яи - V 2“( 2ял)2
But v2» 2 и»/, hence finally
2 л n v ts —————
1.46	Let us take the rotation axis as z-axis whose positive direction is associated with the positive direction of the cordinate cp, the rotation angle, in accordance with the right-hand screw rule (Fig.)
(a) Defferentiating <p (t) with respect to time.
• a - 3 b t2 » ок (1) and dt	x \ /
j2cp d(o
-~^- = M -6bt (2)
dt2 dt	v '
From (1) the solid comes to stop at A t« t»
The angular velocity co = a-3bt\ forOsrsv^b
J (a-3b?)dt	__ .
So, < <o > /	---------- [at-bt3]^a/3b / yfTTSb - 2a/3
Sdt p'
Similarly 0 - | Pz |« 6b t for all values of t.
27
V& fpdt 1 6bt dt .___
So,	---
Sdt
V1
(b) From Eq. (2) pz- -6bt
So,	(PJ - Va73F--6b V^-
H““	p. |(b),_v_|.2VOT
1.47 Angle a is related with | wt | and wn by means of the fomula :
tana - г~\> where wn- <o2R and |wj- 0Я	(1)
I
where R is die radius of die circle which an arbitrary point of the body circumscribes.
From die given equation 0 - ~~  at (here 0 »	’ as 0 is positive for all values of t)
Integrating within the limit£ d<o - a J tdt or, <o - j a r
/ 2	2 4
So,	w2R-I 1 Л-~-R
\ 2 /	4
and	|wr|« РЛ- atR
Putting the values of | wt | and wn in Eq. (1), we get,
a2t*R/4 at3 , Г/41 tan a  ----— - —7— or, t»	— tan a
atR 4	lai
1.48 In accordance with the problem, 0Z < 0
Thus - --7— - к Уш , where к is proportionality constant
When co - 0, total time of rotation t - % 
2V^ к
28
2'/ш0/к
с ( k2t2
I w0 + ~~r~ fa>dt *b \
Average angular velocity < co > = —---» -------------—
’о
Hence < со > «
k2t3 юо' + ЛГ
2V01
Jo
~ %/3
1.49
... К
We have co = co0 - а ф =
Integratin this Eq. within its limit for (ф) t
ф.
а ф r j , co0 - к ф
——= I aZ or, In ---- -kt
,Q и0-*ф J	w0
1.50
Непсе
m Ю° ( 1
(1)
(b) From the Eq., w = ш0-> к ц> and Eq. (1) or by differentiating Eq. (1)
co» co0e
Let us choose the positive direction of z-axis (stationary rotation axis) along the vector In accordance with the equation
d co,	d cor
dt z z dtp z or, coz d coz » d ф « P cos ф d ф, Integrating this Eq. within its limit Ч(ф)
or,
or,
00 sin ф
V 2 0Q sin <p
Hence
The plot co2 (<p) is shown in the Fig. It can be seen that as the angle ф grows, the vector oTfirst increases, coinciding with the direction of the vector (coz > 0), reaches the maximum at <p « qp/2, then starts decreasing and finally turns into zero at ф = л. After that the body starts rotating in the opposite direction in a similar fashion (coz < 0). As a result, the body will oscillate about the position ф « ф/2 with an amplitude equal to Jt/2.
“z“
|
о
2
ф
о
29
Rotating disc moves along the x-axis, in plane motion in x - у plane. Plane motion of a solid can be imagined to be in pure rotation about a point (say 7) at a certain instant known as instantaneous centre of rotation. The instantaneous axis whose positive sense is directed along co of the solid and which passes through the point/, is known as instantaneous axis of rotation.
Therefore lhe velocity vector of an aibitrary point (P) of the solid can be represented as :
(!)
On the basis of Eq. (1) for the С. M. (C) of the disc
w X 7*	(2)
According to the problem vcffi and aTffI*i.e. colx-y plane, so to satisy the —4*
Eqn. (2) rCI is directed along (- j ). Hence point / is at a distance rCI« y, above the centre of the disc along у - axis. Using all these facts in Eq. (2), we get vc
V my or y- —	(3)
(a)	From the angular kinematical equation ®z“ “(te + Pz*
(О « 0Г.
On the other hand x - v t, (where x is the x coordinate of the C.M.)
or,
From Eqs. (4) and (5), co »
(4)
(5)
*
Using this value of co in Eq. (3) we get у « — •
(hyperbola)
(b)	As centre C moves with constant acceleration w, with zero initial velocity
So,
wt
Therefore,
Hence
vc ^2wx
co co
(parabola)
30
132
The plane motion of a solid can be imagined as the combination of translation of the C.M. and rotation about C.M.
So, we may write vA = vc + vA c
- vc + wxrAC	(1) and
6
тттттгпгггтгггтп

»с + ®2(-'кс) + (Гх^с) (2)
rAC is the position of vector of A with respect to C* In the problem vc = v - constant, and the rolling is without slipping i.e., vc » v » co Я, So/ wc = 0 and P = 0. Using these conditions in Eq. (2)
2 wA- ш2(-гас)« <02Я(-иАС)- y(-«AC)
Here, uA c is the unit vector directed along rA c. 2 у	—>	A
Hence wA = — and wA is directed along ( - uA c ) or directed toward the centre of the wheel.
(b) Let the centre of the wheel move toward right (positive x-axis) then for pure tolling on the rigid horizontal surface, wheel will have to rotate in clockwise sense. If co be the vc v
angular velocity of the wheel then co « — » —. К к
Let the point A touches the horizontal surface at t« 0, further let us locate the point A at t  /, When it makes 0 « co t at the centre of the wheel.
From Eqn. (1)	vj + uTx
» v i + co (- k) x [J? cos 0 (- j ) + /? sin 0 (- i) ] >	—►	—>	—>
or,	vA » v i + co R [ cos co t i) + sin art j ]
—4»	—>
» (v - cos co/) i + v sin co/ j (as v « co/?)
So, vA » V (v - v cos co/)2 + (v sin co/)2-
= vV2(l - cosco/) « 2vsin(co//2)
Hence distance covered by the point A during T» 2 л/со
2 я/ш
8v vAd/» I 2 v sin(co//2) dt« — » SR.
о
1.53 Let us fix the co-ordinate axis xyz as shown in the fig. As the ball rolls without slipping along the rigid surface so, on the basis of the solution of problem 1.52 :
vn«v^ + coxr*»0
Thus	„ 0 .	CO
vc » inR and co у у (- k) as vc у f i
31
.	®с+Рхг«^°
wc- PR and P*f t (- к) as й£ f J i
At the position corresponding to that of Fig., in accordance with the problem, we« w, so vc« wt
A Vc+^=VA
V- \AJt	W
and co - —and P - — (using 1) К	К	К
(a) Let us fix the co-ordinate system with the frame ГПТГП/
attached with the rigid surface as shown in the Fig.
As point О is the instantaneous centre of rotation of the ball at the moment shown in Fig. so,
vj- О,
Now,	vA « vc + co x rAC
» vc i + co (- к ) x R (j ) - (vc + соЛ) i
So,	vA « 2 vc i  2 wt i (using 1)
> _i >
Similalry vB - vc + co x  vc i + co (- к ) x R (i)
- vci + a>R(-j)- vci + vc(-})
So, vB « V2 vc » V2 wt and vB is at an angle 45° from both i and j (Fig.)
(b) 4“ "£ + <»2(-0 + i5*'oc 2	— VC л
- “ (- roc) “ у (- uoc) (usin8 where is the unit vector along
so, H>0 - — - —— (using 2) and w0 is К К
directed towards the centre of the ball
Now wA - wc + <o2 (- r^c) + P*x i^c
« w i + <o2 R (- j) + P (- к ) x R j
(w + РЯ ) i +	(- j ) (using 1)
A
n
So,
Similarly
й^- й£ + w2(-r^.) + P*x r^.
 wi + a>2R (- i) + p (- к) x R (i)
v-p + PR(-j) (using 1) A /
32
1.54
1.55
So,
'	w2,2}
w - —- i + w (-j) (using 2) л I

12
+ w2
Let us draw the kinematical diagram of the rolling cylinder on the basis of the solutioi of problem 1.53.
0
As, an arbitrary point of the cylinder follows a curve, its normal acceleration and radius of curvature are related by the well known equation
so, for point A,
or,
Similarly for point Д
or,
W”~ R
Ra - —y « 4r (because ve •= <or, for pure rolling)
’ r;
2	4,o (^2\)2
co r cos 45 = —--,
RB
R„- 2V2 —x-« 2V2r B co2r
The angular velocity is a vector as infinitesimal rotation commute. Then die relative angular velocity of the body 1 with respect to the body 2 is clearly.
—►	—-> —>
as for relative linear velocity. The relative acceleration of 1 w.r.t 2 is
( dt
's'
33
where S' is a frame corotating with the second body and 5 is a space fixed frame with origin coinciding with the point of intersection of the two axes,
but
+ C02 X COj
• )
Since S rotates with angular velocity w2 . However j » 0 as the first body rotates
with constant angular velocity in space, thus
x <0^.
Note that for any vector FT the relation in space forced frame (k) and a frame (£') rotating with angular velocity coTis
dt
d# dt
+ ю x F*
к
1.56 We have co = at i + bt2j
So, со» V(a/)2 + (bf2)2 , thus, co|r- 10s» 7.81 rad/s
Differentiating Eq. (1) with respect to time d CO т-> л p -	» ai + 2btj
(1)
(2)
So,
and
(b)
0- V a2 + (2 bt)2 PL-ю. - 1-3rad/s2
coSaw	(a‘i + bt2j )-(ai + 2btj )
“P V (at)2 + (for2)2 V л2 + (2bt)2
Putting the values of (a) and (b) ^hd' taking t« 10s, we get a- 17°
1.57 (a) Let the axis of the cone (ОС) rotates in anticlockwise sense with constant angular velocity a? and the cone itself about it’s own axis (ОС) in clockwise sense with angular velocity (Fig.). Then the resultant angular velocity of the cone.
co - co +co0	(1)
As the rolling is pure the magnitudes of the vectors and co0 can be easily found from Fig.
co'» p —. , co0- v/R (2) R cot a u	'
As co* 1 co^, from Eq. (1) and (2)
34
О) - V О)'2 + О>0
- / / V V / V V V V 7— + Ы - п-------------------------- 2-3 rad/s
¥ IR cot a I	IRI	R cos a
(b) Vector of angular acceleration
— du d(w' + w0) P » T e ---------з------- (as a) « constant.)
r dt dt	f
The vector (o0 which rotates about the OO' axis with the angular velocity of, retains i magnitude. This increment in the time interval dt is equal to
I d co01 - (o0- co' dt or in vector form d co0 - (<o x co0) dt.
Thus	(o’ xcoj,	(3
The magnitude of the vector (5* is equal to p « (o' (o0 (as ш ± (0^)
v v v2
So,	p « ——-— — « —г tan a * 2-3 rad/s
Я cot а Я R2
1.58 The axis AB acquired the angular velocity
W	0A
Using the facts of the solution of 1.57, the	< A v
angular velocity of the body co » V(o2 + co'2
- Vu>o + pj;t2 = 0-6 rad/s
And the angular acceleration.
Д. du d(<a+m0) dt “ dt
d(Q0 _doo
But	 . - to x<on, and —;—
dt	°’ dt
0/=Л t
аГо A
dt +~dT
Po*
So,	?*« (j^rx +
As, Pjj 1 <о^ so, P *	(<o0 Po t)2 + рд « po V 1 + (<o01)2 - 0-2 rad/s2
35
12 THE FUNDAMENTAL EQUATION OF DYNAMICS
1.59	Let R be the constant upward thurst on the aerostat of mass m9 coming down with a constant acceleration w. Applying Newton’s second law of motion for the aerostat in projection form
Fy ж mwy
mg-R- mw
Now, if Am be the mass, to be dumped, then using the Eq. Fy - mwy R-(m- Am) g- (m- Am) w,
From Eqs. (1) and (2), we get, Am «
(1)
(2)
1.60	Let us write the fundamental equation of dynamics for all the three blocks in terms of projections, having taken the positive direction of x and у axes as shown in Fig; and using the fact that kinematical relation between the accelerations is such that the blocks move
with same value of acceleration (say и»)
mog-7’1-C1)
Tx - T2 - ктг g « mr w	(2)
and T2-km2g» m2w	(3)
The simultaneous solution of Eqs. (1), (2) and (3) yields,
+	]	.J
w- g--------------- 41
m0 + mx + m2	6 ’
and
(l+*)m0
2 m0 + тх + m2 &
As the block mQ moves down with acceleration w, so in vector form _ [m0 - к (mj + mj) ] g* w* -----------------------------------------------
m0 + mx + m2
1.61	Let us indicate the positive direction of x-axis along the incline (Fig.). Figures show the force diagram for the blocks.
Let, R be the force of interaction between the bars and they are obviously sliding down with the same constant acceleration w.
36
Newton’s second law of motion in projection form along x-axis for the blocks gives :
mr g sin a - kY g cos a + R * mr w	(1)
m2 g sin a - R - J^ m2 g cos a - m2 w	(2)
Solving Eqs. (1) and (2) simultaneously, we get
k1m1’¥k2m2 w = g sin a - g cos a---------and
+ m2
R_	cos a
mY + m2
(b) when the blocks just slide down the plane, w - 0 , so from Eqn. (3)
mi + ^2 ^2 rsina-ecosa---------------« 0
mr + m2
or, (mr + since»	m2) cos a
(JL + k, m0)
Hence	tan a - 1 1	- —
mY + m2
1.62 Case 1. When the body is launched up :
Let к be the coefficeint of friction, и the velocity of projection and I the distance traversed along the incline. Retarding force on the block - mg sin a + к mg cos a and hence the retardation - g sin cl + kg cos a.
Using the equation of particle kinematics along the incline, 0« M2-2(gsina + fcgcosa)Z
2
or,	^7~--------4----------7	(1)
2 (g sin a + kg cos a)	'
and	0- u-(gsina + fcgcosa)r
or,	и - (g sin a + kg cos a) t	(2)
1	2
Using (2) in (1) I - у (g sin a + kg cos a) t	(3)
Case (2). When the block comes downward, the net force on the body - mg sin a - km g cos a and hence its acceleration « g sin cl - к g cos a Let, t be the time required then,
1	2
/= — (g sin a-Jig cos a) f	(4)
From Eqs. (3) and (4) ? sin a-A: cos a f 2 sin a + к cos a
But у «	(according to the question),
Hence on solving we get
Jl = tan a - 0-16 (T|2 + l)
37
1.63	At the initial moment, obviously the tension in the thread connecting ml and m2 equals the weight of
(a)	For the block m2 to come down or the block m2 to go up, the conditions is
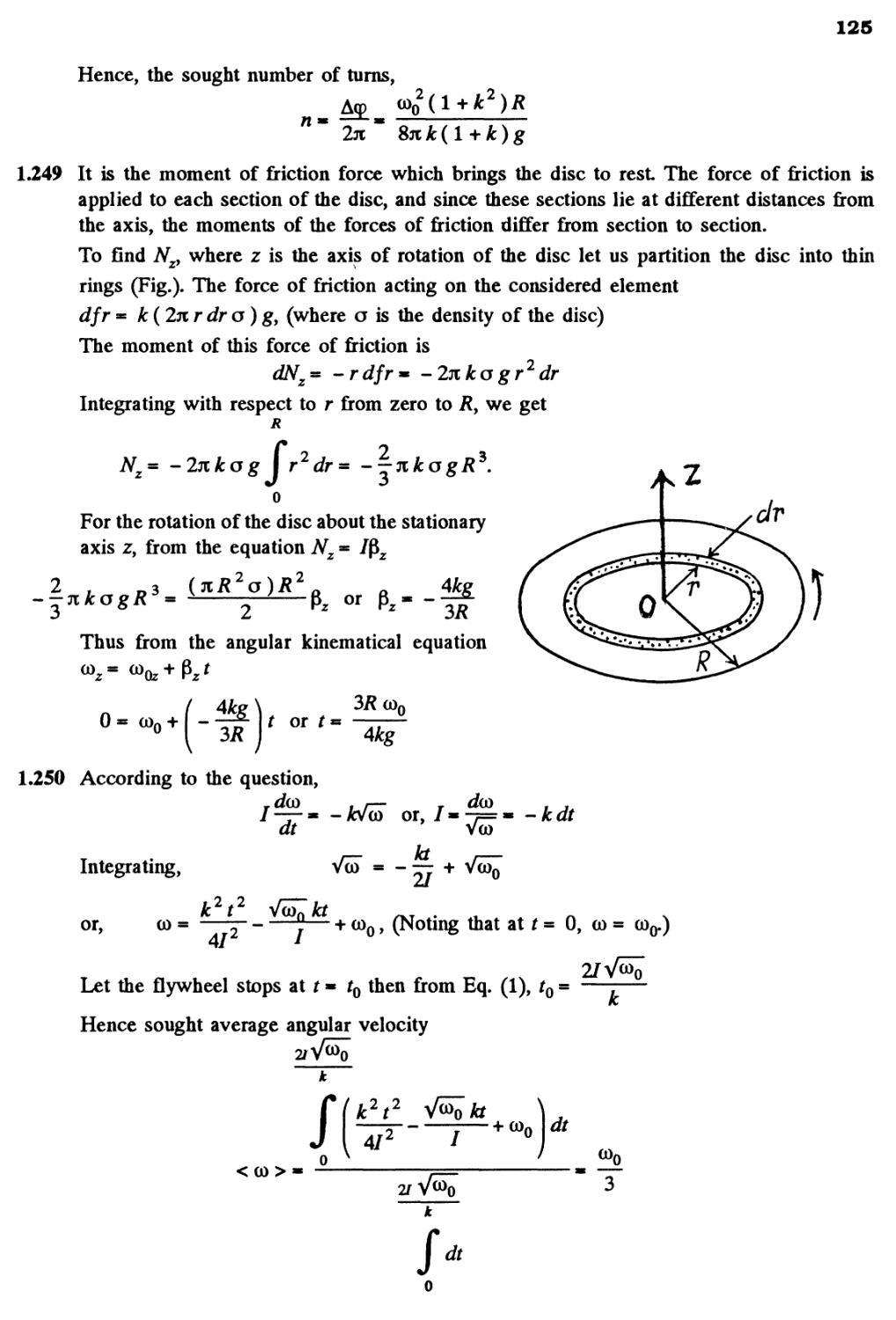
m2 S “ T * 0 and T - mY g sina - fr г. 0
where T is tension and fr is friction which in the limiting case equals кт$ cosa. Then
or m2g- z^sina > km1gcos a
m2
or	— > (k cos a + sin a)
(b)	Similarly in the case
or, m1gsina-m2g>km1gcosa
m2
or,	— < (sin a - к cos a)
wi
(c)	For this case, neither kind of motion is possible, and fr need not be limiting.
m2
Hence,	(k cos a + sin a) > — > (sin a - к cos a)
1.64	From the conditions, obtained in the previous problem, first we will check whether the mass m2 goes up or down.
Here, n^/m!« t] > sin a + к cos a, (substituting the values). Hence the mass m2 will come down with an acceleration (say w). From the free body diagram of previous problem, w2-g-T-w	(1)
and	T - mx g sin a - kmxg cos ex ~ w	(2)
Adding (1) and (2), we get, m2 g - mx g sin a - к mx g cos a - (mx + m2) w
_ (m2/m1-sina-kcosa)g (n-sma-jfccosct)g
W ~	(1 + Г^/ГП])	Ж	1 + T|
Substituting all the values, w - 0-048 g * 0-05 g
As m2 moves down with acceleration of magnitude w « 0.05 g > 0, thus in vector form acceleration of m2:
0 05-
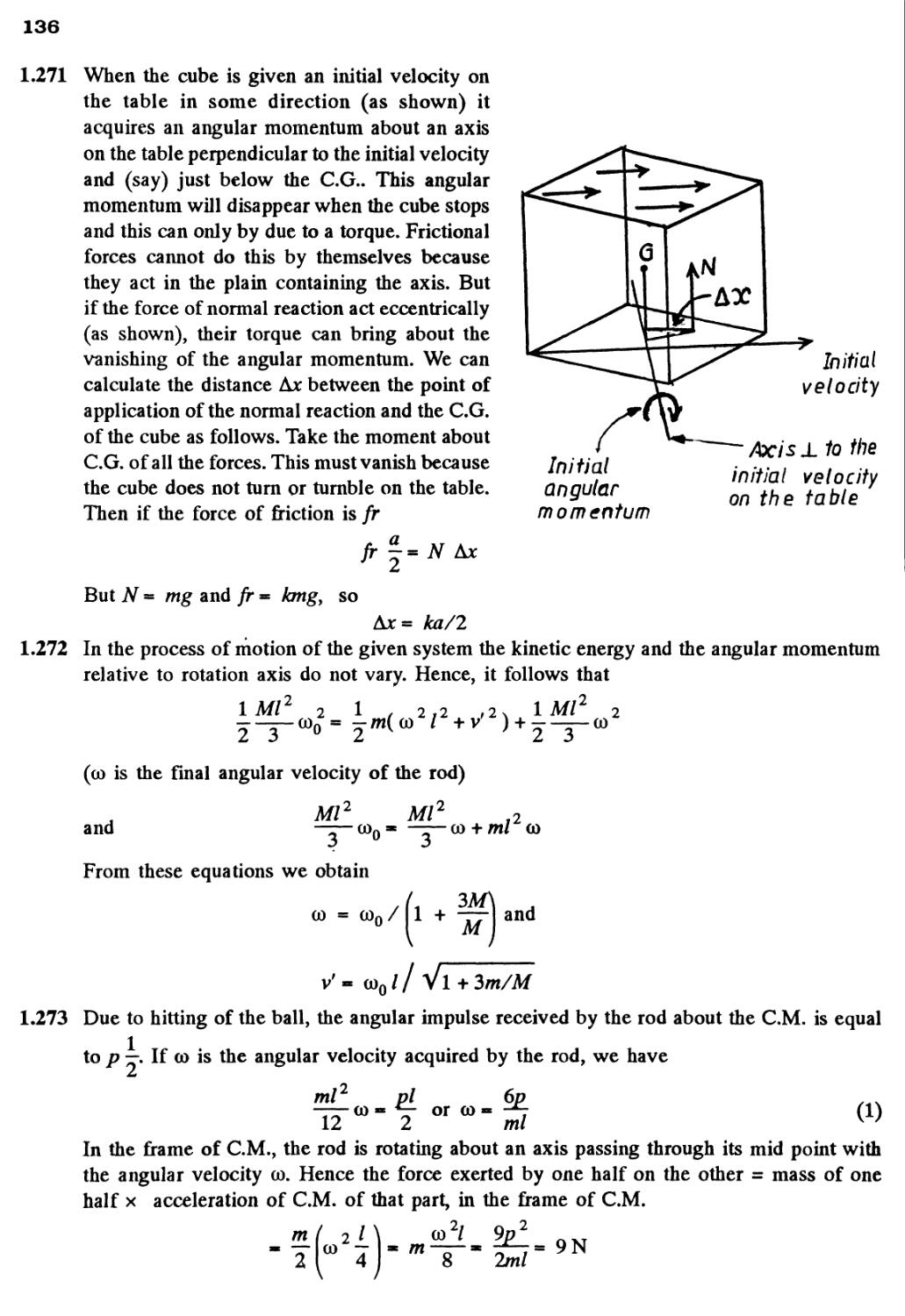
1.65	Let us write the Newton’s second law in projection form along positive x-axis for the plank and the bar
fr « m1w19 fr» m2 w2
(1)
38
At the initial moment, fr represents the static friction, and as the force F grows so does the friction force fr, but up to it’s limiting value i.e./r=	kN= km2g.
Unless this value is reached, both bodies moves as a single body with equal acceleration. But as soon as the force fr reaches the limit, the bar starts sliding over the plank i.e. w2 * wr
77777777777777777777ТШ7
Substituting here the values of and w2 taken from Eq. (1) and taking into account that
Jtm2
/г« к m2 g, we obtain, (at~km2g)/m2*-----g, were the sign "=" corresponds to the moment
t- t0 (say)
Hence,
If
kgm2(m1 + (l m^
km2g
t £ r0, then wt« ---(constant), and
w2 = (at-km2g)/m2
On this basis (r) and w2 (r), plots are as shown in the figure of answersheet.
1.66	Let us designate the x-axis (Fig.) and apply Fx = m wx for body A :
mgsin a - Armgeos a = mw
or,	w = g sin a - к g cos a
Now, from kinematical equation :
/ sec a « 0 + (1/2) w t2 t- V2l sec a/(sin a - Arcos a) g - V 2 I /(sin 2 a/2 - £ cos2 a) g
or,
(using Eq. (1)).
. ( sin 2 a .	2 '
a —-— - к cos a
for t • , min r
da
i.e.
2 cos 2 a	л
---------+ 2 к cos a sin a - 0
- 0
or,
tan 2 a * - -7 => a = 49’ к
and putting the values of a, к and I in Eq. (2) we get - Is.
1.67	Let us fix the x -y co-ordinate system to the wedge, taking the x - axis up, along the incline and the у - axis perpendicular to it (Fig.).
39
Now, we draw the free body diagram for the bar.
Let us apply Newton’s second law in projection form along x and у axis for the bar : T cos p - m g sin a - fr = 0	(1)
Tsin p+JV-mgeos a = 0
or, mgcosa-Tsinp	(2)
But fr~ kN and using (2) in (1), we get
T- m gsin a + к mg cos a/(cos P + JI sin P)
For the value of (cos P + fcsin P) should be maximum
_	J (cos P + fcsin p) л	t
So,	—1- 0 or tan P - £
“P
Putting this value of P in Eq. (3) we get, T mg(sina + £cos a) m g (sin a + Jfccos a)
" 1 / Vl+fc2 + k2 / Vl +i2	Vl+Jt2
1.68	First of all let us draw the free body diagram for the small body of mass m and indicate x - axis along the horizontal plane and у - axis, perpendicular to it, as shown in the figure. Let the block breaks off the plane at t« tQ i.e. N « 0
So, mg- arosina * 0
From Fx « mwx, for the body under investigation :
m d y/dt« ar cos a ; Integrating within the limits for v (r)
m
о
о
So,
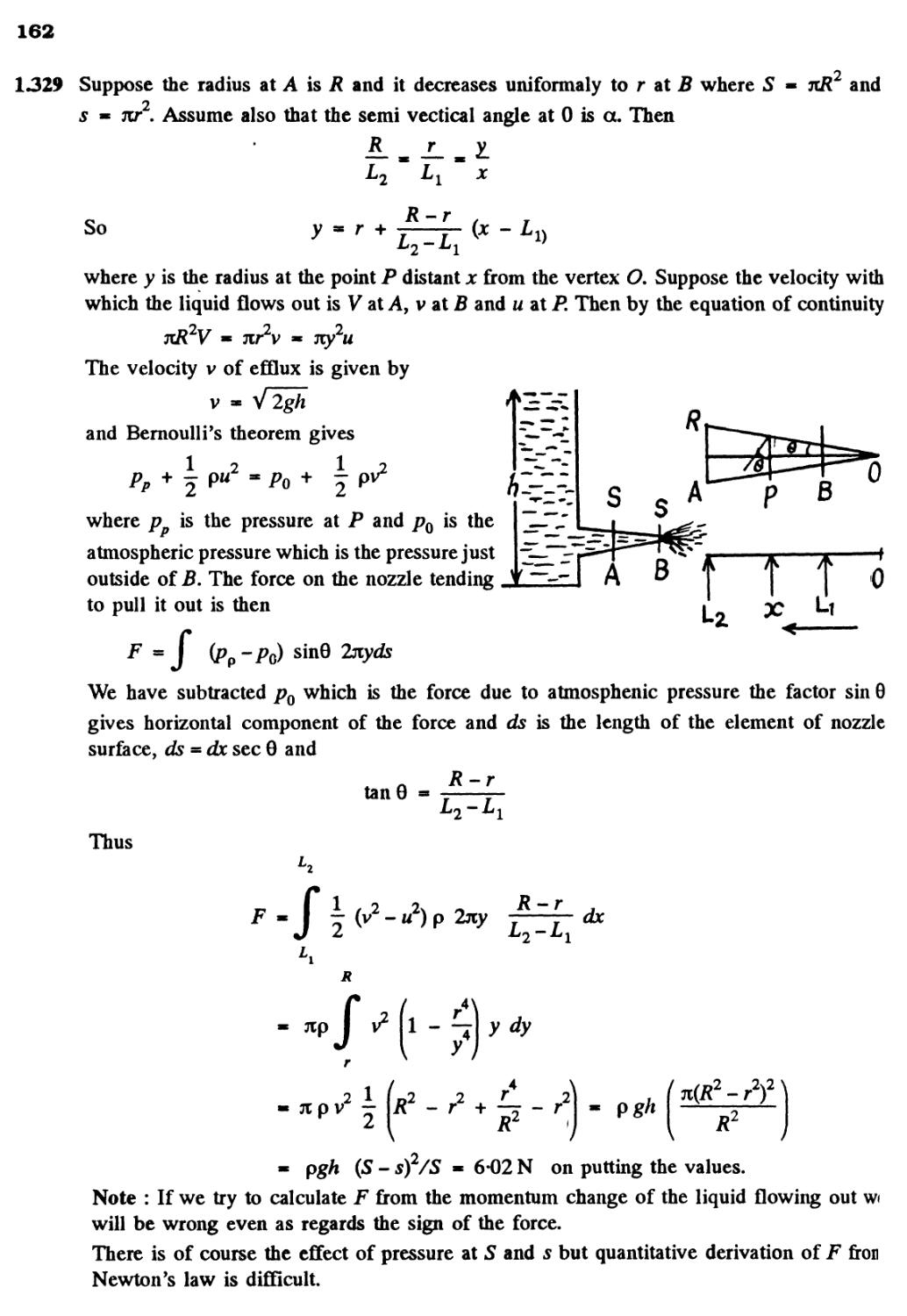
ds a cos a 2
dt 2m f
(2)
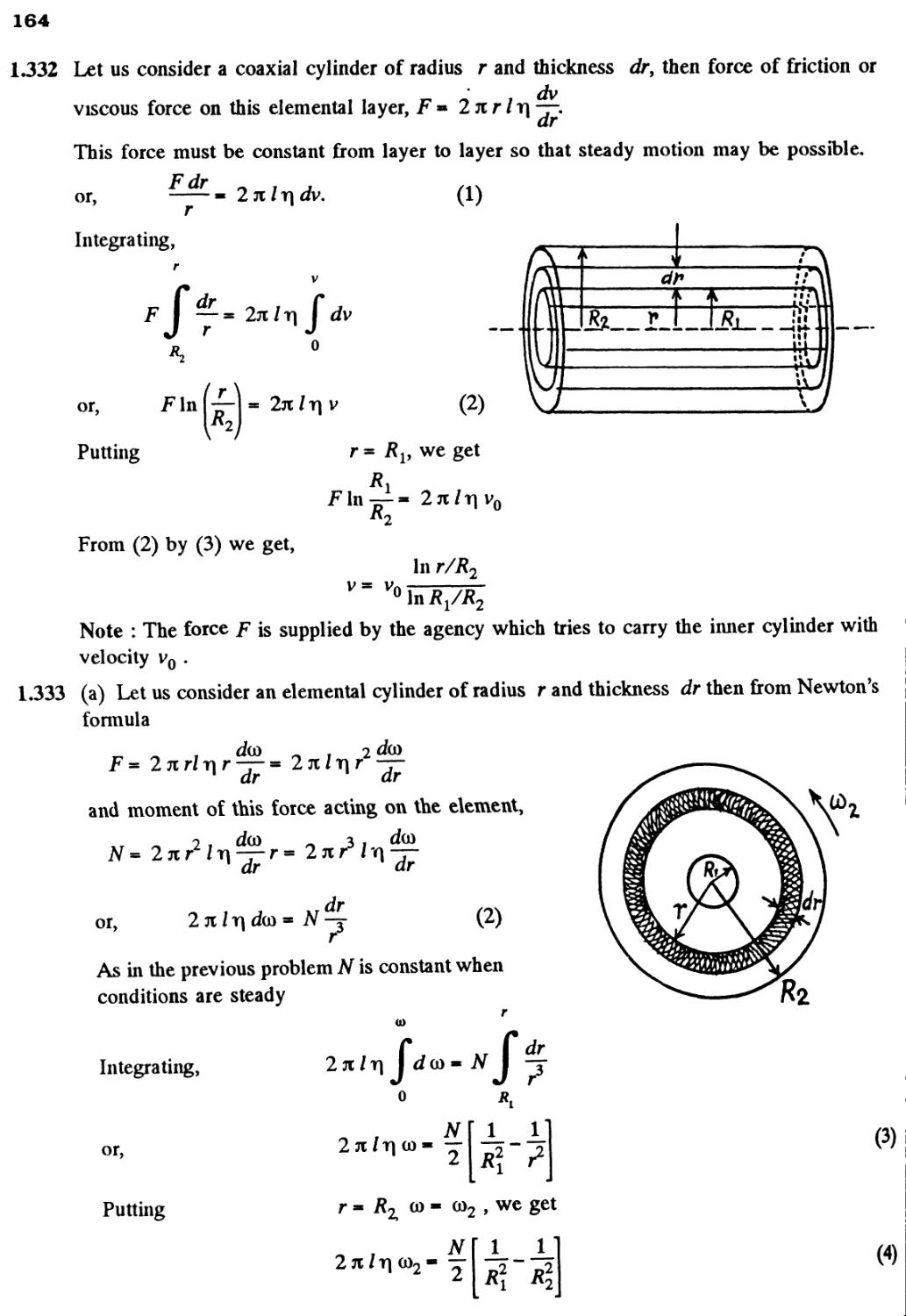
v
Integrating, Eqn. (2) for s (t)
S’ -
3 a cos a r
2m 3
(3)
Using the value of t« t0 from Eq. (1), into Eqs. (2) and (3)
m g2 cos a . m2 g3 cos a v« —й—z— and	“—z—
2	a sin a	6 a sin a
40
1.69
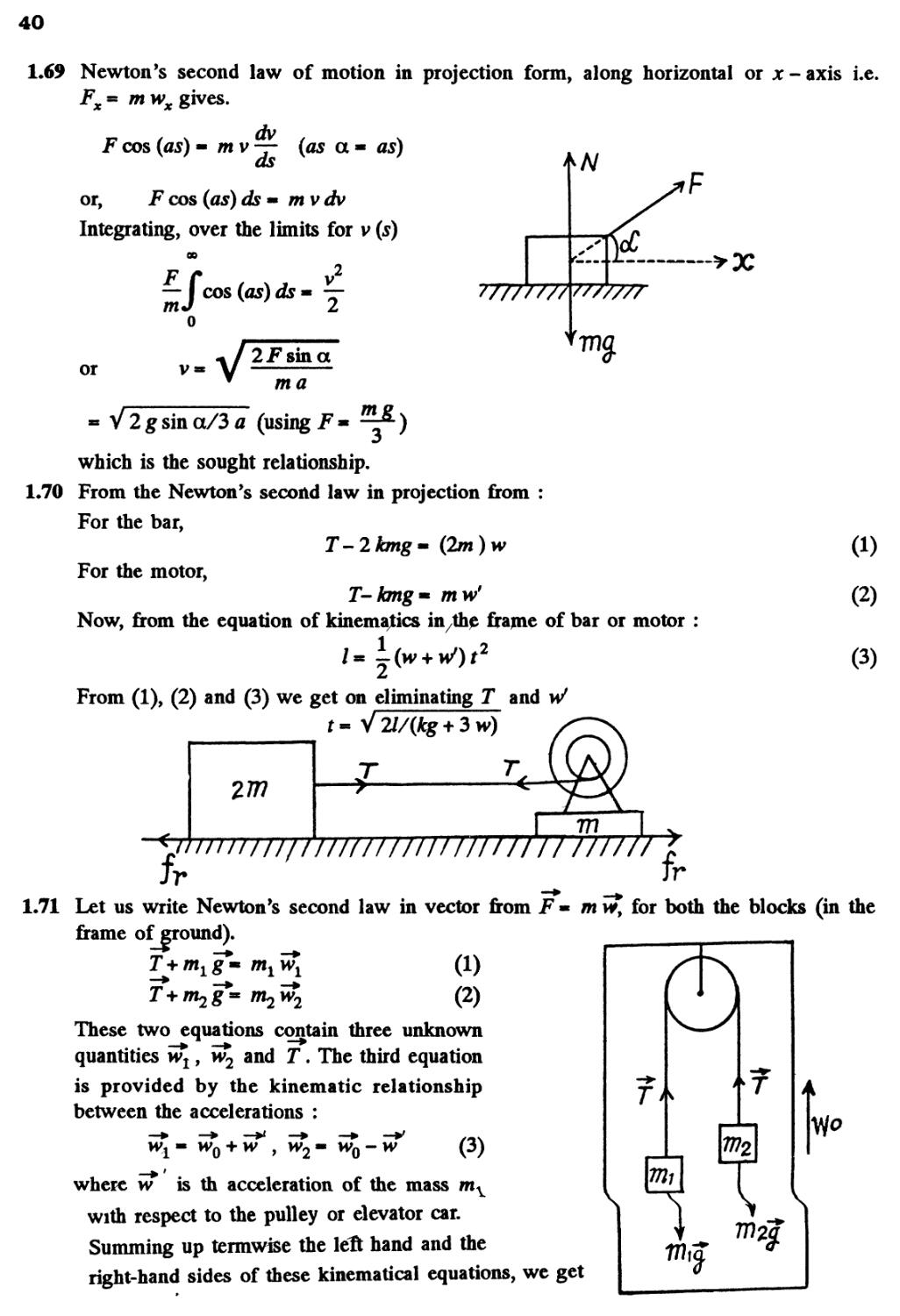
Newton’s second law of motion in projection form, along horizontal or x - axis i.e. Fx = m wx gives.
F cos (as) ~	(as a « as)
as
or, Feos (as) ds- mvdv
Integrating, over the limits for v (5)
2
1.70
mJ 0
V2Fsina
— ma
V 2 g sin a/3 a (using F-which is the sought relationship. From the Newton’s second law in projection from : For the bar,
or
T - 2 kmg - (2m) w
1.71
For the motor,
(1)
T-kmg- mw'
Now, from the equation of kinematics inthe frame of bar or motor : /ж 1(w + m/)/2
From (1), (2) and (3) we get on eliminating T and и/ r- V27/(lg + 3w)
2777
>
I 777 I
^}пп1)}11ГП1/1Н/1ПН'ГГГ1П'/11П7'^
(2)
(3)
Let us write Newton’s second law in vector from F * m w, for both the blocks (in the frame of^round).
T+^g*-
(1) (2) These two equations contain three unknown quantities Ц, w2 and T. The third equation is provided by the kinematic relationship between the accelerations :
- WO + w , W2 - w0 - w (3) where w is th acceleration of the mass mv with respect to the pulley or elevator car. Summing up termwise the left hand and the right-hand sides of these kinematical equations, we get
41
+	2w0
(4)
The simultaneous solution of Eqs*(l), (2) and (4) yields
->	(»»! - m2) g + 2 m2 w0
------------------------
m1+ m2
Using this result in Eq. (3), we get,
m. -	¥
w =
and
-> 2 m.	.
Г= m + ^2
Using the results in Eq. (3) we get
W ~ m + m ~ m1^m2
(b) obviously the force exerted by the pulley on the celing of the car
F- -2T--------— (F~^o)
ml + m2
Note : one could also solve this problem in the frame of elevator car.
1.72 Let us write Newton’s second law for both, bar 1 and body 2 in terms of projection having taken the positive direction of and x2 as shown in the figure and assuming that body 2
starts sliding, say, upward along the incline
Л “ mi S s^n a = miwi	(1)
m2g-T2= m2w	(2)
For the pulley, moving in vertical direction from the equation Fx « m wx
2T2-Tl = (mp)wr - 0
(as mass of the pulley mp « 0)
or	T\« 2 T2	(3)
As the length of the threads are constant, the kinematical relationship of accelerations becomes

w=2w1	(4)
Simultaneous solutions of all these equations yields :
w ®
2 g (2 T] - sin a) (4t] + 1)
As T] > 1, w is directed vertically downward, and hence in vector form
-» 2 g*(2 T] - sin a)
4r| + l
42
1.73 Let us write Newton’s second law for masses and m2 and moving pull у in vertical direction along positive x - axis (Fig.) :
(1)
m2g-T=m2w2x	(2)
Tx - 2T = 0 (as m = 0)
or	T\ - 2 T	(3)
Again using Newton’s second law in projection form for mass m0 along positive xr direction (Fig.), we get
ri“mowo	(4)
The kinematical relationship between the accelerations of masses gives in terms of projection on the x - axis
- 2 w0	(5)
Simultaneous solution of the obtained five equations yields : _ [4m1m2 + m0(m1-m2)]g
1	4т1т2 + т0 (mt + m^)
In vector form
_	[4	*”2 + w0 (wi ~ >”2) ] g
1	4m1m2 + m0 (m1 + mJ
1.74 As the thread is not tied with m, so if there were no friction between the thread and the ball m, the tension in the thread would be zero and as a result both bodies will have free fall motion. Obviously in the given problem it is the friction force exerted by the ball on the thread, which becomes the tension in the thread. From the condition or language of the problem wM > wm and as both are directed downward so, relative acceleration of M = wM - wm and is directed downward. Kinematical equation for the ball in the frame
of rod in projection form along upward direction gives
(1)
Newton’s second law in projection form along vertically down direction for both, rod and ball gives,
Mg-fr-Mw„	(2)
mg-fr- mwm	(3)
Multiplying Eq. (2) by m and Eq. (3) by M and then subtracting Eq. (3) from (2) and after using Eq. (1) we get . 2lMm 1Г~ (M-m)t2
I
43
1.75 Suppose, the ball goes up with accleration and the rod comes down with the acceleration w2.
As the length of the thread is constant, 2	= w2	(1)
From Newton’s second law in projection form along vertically upward for the ball and vertically downward for the rod respectively gives,
T - m g « m	(2)
and Mg-T’-Mw2	(3)
but	T = 2 T (because pulley is massless)
From Eqs. (1), (2), (3) and (4)
(2M -m)g (2 - тр g	.	. .
W1 = m + AM S r| + 4 Ш uPward direction)
2 (2 - n) g
and w2 =	4) (downwards)
From kinematical equation in projection form, we get
l~ ^(wl + w2)t2
as, and w2 are in the opposite direction.
Putting the values of and w2, the sought time becomes
(4)
t = V 2 / (t| + 4) / 3 (2 - T]) g = 1-4 s
1.76 Using Newton’s second law in projection form along x - axis for the body 1 and along negative x - axis for the body 2 respectively, we get mlg-Tl-mlw1	(1)
T2-m2g~m2w2	(2)
For the pulley lowering in downward direction from Newton’s law along x axis,
I\ - 2 T2 e 0 (as pulley is mass less)
or,	Tj = 2 T2	(3)
As the length of the thread is constant so,
И72= 2wi	(4)
The simultaneous solution of above equations yields, 2 (mj - 2m2) g 2 (ti - 2) = ---------------------------------« —~
2	4 m2 +	T| + 4
ml
(as — = n) m2
/7777777777777
(S)
Obviously during the time interval in which the body 1 comes to the horizontal floor covering the distance A, the body 2 moves upward the distance 2h. At the moment when the body 2 is at the height 2h from the floor its velocity is given by the expression :
v\ - 2 w2 (2h) - 2
•2(T]-2)g'
T| + 4
2/,-. 8/t(n-2)g T| + 4

After the body touches the floor the thread becomes slack or the tension in the thread zero, thus as a result body 2 is only under gravity for it’s subsequent motion.
44
Owing to the velocity v2 at moment or at the height 2Л from the floor, the body 2
further goes up under gravity by the distance,
4Л(т|-2) 2g “ т] + 4
Thus the sought maximum height attained by the body 2 :
1J7
H- 2h + h'- 2h +	-
0l + 4)
6т)Л
T) + 4
Let us draw free body diagram of each body, i.e. of rod A and of wedge В and also draw the kinemetical diagram for accelerations, after analysing the directions of motion of A and B. Kinematical relationship of accelarations is :
tana « —	(1)
Let us write Newton’s second law for both bodies in terms of projections having taken positive directions of у and x axes as shown in the figure.
mA g-Ncos a - mA wA	(2)
and	N sin a = mBwB	(3)
Simultaneous solution of (1), (2) and (3) yields :
mAgsina	g
w - ------------------------- ----S------ and
л mA sm a + mB cot a cos a (1 + cot2 a)
wa	g
И>я = ---« 7-------2-------г
° tan a (tan a + T) cot a)
Note : We may also solve this problem using conservation of mechanical energy instead of Newton's second law.
1.78	Let us draw free body diagram of each body and fix the coordinate system, as shown in the figure. After analysing the motion of M and m on the basis of force diagrams, let us draw the kinematical diagram for accelerations (Fig.).
As the length of threads are constant so,
ж and as vmM an^ VM not c^ange their directions that why
I = I “ w (say) and "Lf tt and WM ft
45
AS Wm = WmM+WM
so, from the triangle law of vector addition
wM-V2w	(1)
From the Eq. Fx - m wx , for the wedge and block :
T-N~Mw,	(2)
and	N- mw	(3)
Now, from the Eq. Fy « mwy, for the block
mg — T — kN = mw	(4)
Simultaneous solution of Eqs. (2), (3) and (4) yields :
----”g ..---------8.------
(Лт + 2т+Л/)	(£ + 2+M/m)
Hence using Eq. (1)
________£2^-----
" (2 + k + M/m)
1.79	Bodies 1 and 2 will remain at rest with repect to bar A for ws и> s и> , where wminis л	min	mfly?	min
the sought minimum acceleration of the bar. Beyond these limits there will be a relative motion between bar and the bodies. For 0 £ w £ и^п, the tendency of body 1 in relation to the bar A is to move towards right and is in the opposite sense for w £ w^. On the basis of above argument the static friction on 2 by A is directed upward and on 1 by A is directed towards left for the purpose of calculating w^.
Let us write Newton’s second law for bodies 1 and 2 in terms of projection along positive
x - axis (Fig.).
T-fr^ mw or, T-mw	(1)
N2- mw	(2)
As body 2 has no acceleration in vertical direction, so
A2=* mg-T (3)
From (1) and (3) (^i+A2)e	(4)
But fr1^fr2^k(N1^N2)
or Ai + fr2 £ к (mg + mw) (5)
46
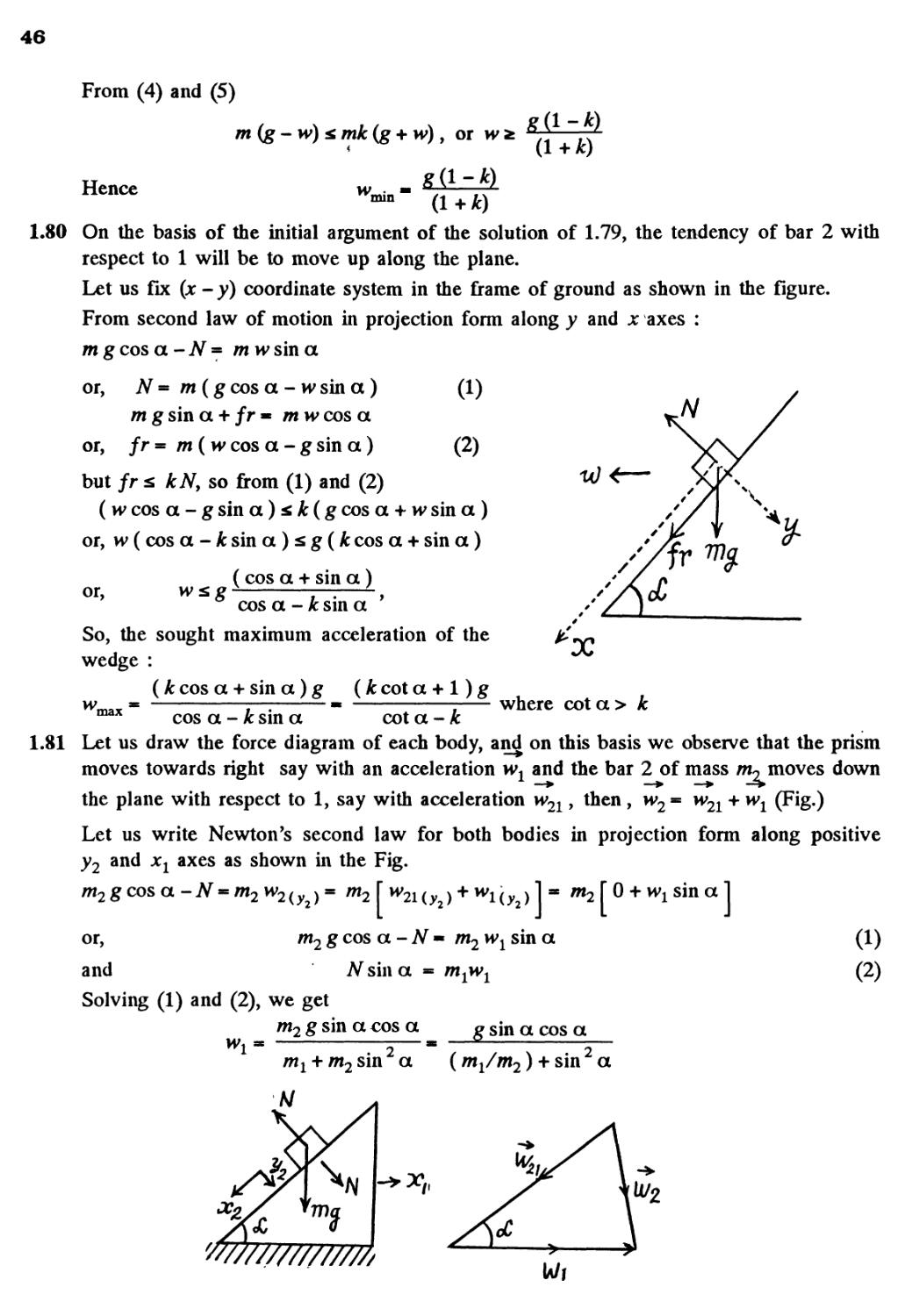
Непсе
1.80
From (4) and (5)
m(g~w)*mk(g + w), or wt “—it2-<	(1 + k)
nun (1 + fc)
On the basis of the initial aigument of the solution of 1.79, the tendency of bar 2 with
respect to 1 will be to move up along the plane.
Let us fix (x - y) coordinate system in the frame of ground as shown in the figure.
From second law of motion in projection form along у and x axes :
m g cos a - N = m w sin a
or, N = m(g cos a - w sin a )	(1)
m g sin a + fr « mw cos a
or, f r = m ( w cos a - g sin a)	(2)
but fr x kN, so from (1) and (2)
( w cos a-gsina)^i(g cos a + w sin a ) or, w ( cos a-fcsina)=sg(fc cos a + sin a)
( cos a + sin a ) or, W £ g  ------------;—;---- ,
cos a - к sin a
So, the sought maximum acceleration of the wedge :
(к cos a + sin a) g (к cot a + 1) g
_ = -----------—-----w --------------where cot a > к
cos a -1 sm a cot a - к
1.81	Let us draw the force diagram of each body, and on this basis we observe that the prism moves towards right say with an acceleration wr and the bar 2 of mass	moves down
the plane with respect to 1, say with acceleration w21, then , w2 = w21 +	(Fig.)
Let us write Newton’s second law for both bodies in projection form along positive y2 and xr axes as shown in the Fig.
zrz2 g cos oe-N = zn2 w2(yp = m2 [ ^2i(y2)+ wi(y2) ] “	[ 0 + sin a ]
or,	m2 g cos a - N - m2 sin a	(1)
and	jV since =	(2)
Solving (1) and (2), we get
m2 g sin a cos a g sin a cos a
“ ------------2---“ ---&----------2~
mY + m2 sin a (mY/m2) + sin a
47
1.82	To analyse the kinematic relations between the bodies, sketch the force diagram of each body as shown in the figure.
On the basis of force diagram, it is obvious that the wedge M will move towards right and the block will move down along the wedge. As the length of the thread is constant, the distance travelled by the block on the wedge must be equal to the distance travelled by the wedge on the floor. Hence dsmM - ds^. As and do not change their directions and acceleration that’s why tt and tt an<^ =	= w
(say) and accordingly the diagram of kinematical dependence is shown in figure.
As wm «	+ wM, so from triangle law of vector addition.
Wm =	WmM WMC0S “ ~ W^2^ ~ 008 “)	C1)
From Fx = m wx, (for the wedge),
T = Tcos a + jVsin a « Mw	(2)
For the bar m let us fix (x -y) coordinate system in the frame of ground Newton’s law in projection form along x and у axes (Fig.) gives
mg since - T - m wm w- m (x)+ wM (x)l
= m + V0S (Я ~ a)] “ m W (1 “ COSa) (3) mgcosa-N- mwm{y}- m [ wmM(y) + wM{y) ] - «[0 + wsina]	(4)
Solving the above Eqs. simultaneously, we get m g sin a
M + 2m (1 - cos a )
Note : We can study the motion of the block m in the frame of wedge also, alternately we may solve this problem using conservation of mechanical energy.
1.83	Let us sketch the diagram for the motion of the particle of mass m along the circle of radius R and indicate x and у axis, as shown in the figure.
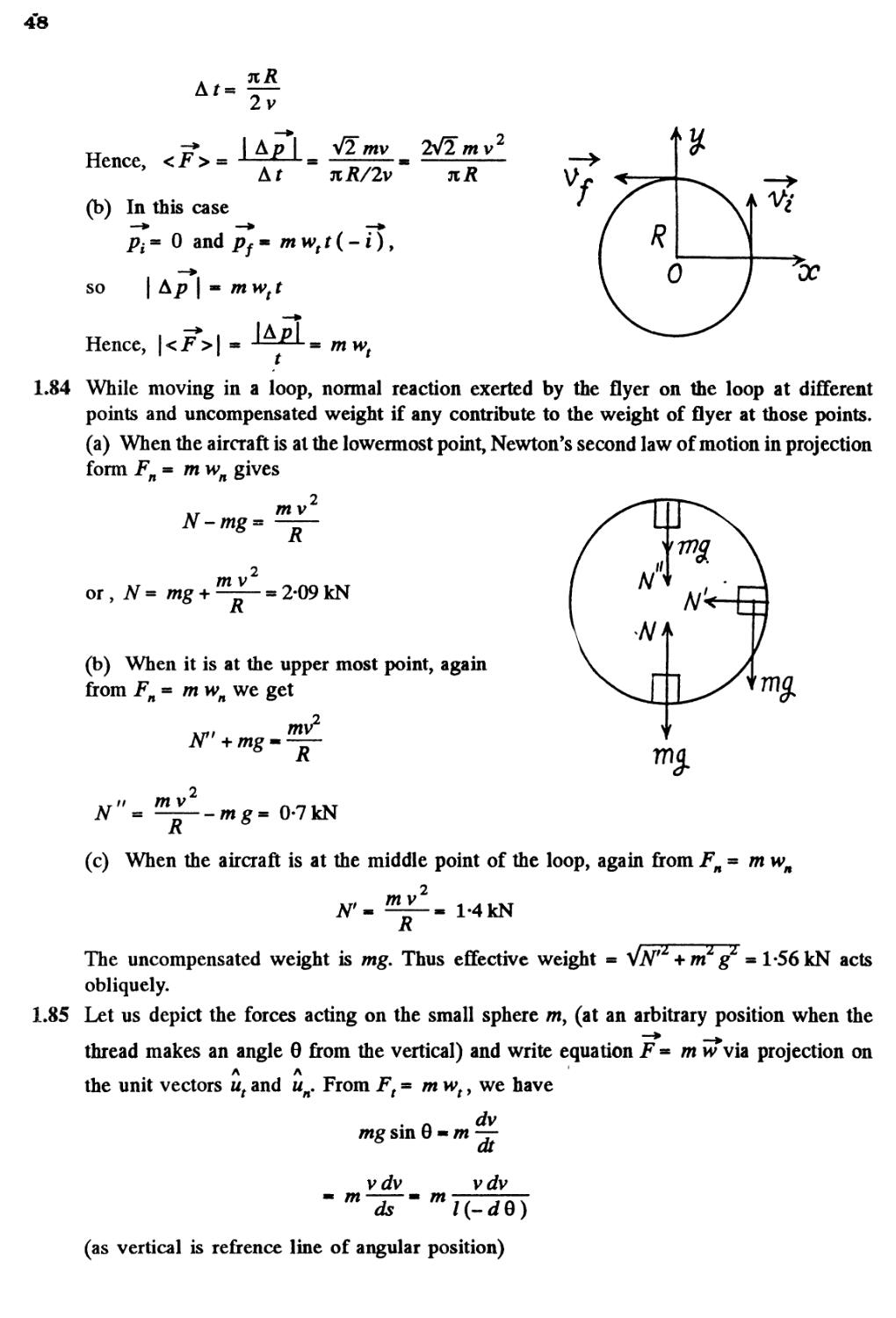
(a)	For the particle, change in momentum Ap « mv (- i) - m v (j)
so, | Ap | « m v
and time taken in describing quarter of the circle,
48
A t -
jiR
2v
tt	z? Ap	V2 mv	2^2 mv2
Hence, <F>= -1—x—*- = —--------------------—
Ar nR/2v nR
(b)	In this case —>	—->	—>
p- = 0 and py«
so | Ap | “ m wt t
—->
Hence, I < F > I =	= m wt
1	1 t	1
1.84 While moving in a loop, normal reaction exerted by the flyer on the loop at different points and uncompensated weight if any contribute to the weight of flyer at those points, (a) When the aircraft is al the lowermost point, Newton’s second law of motion in projection form Fn = mwn gives
	2 * г	V
N-mg =
m у 2
or, N= wg + ^- = 2*09kN R
(b) When it is at the upper most point, again
from Fn = m wn we get
КГ»
№ +mg- —
N"-	o-7kN
R
(c) When the aircraft is at the middle point of the loop, again from FH = m wn
N'-	1-4kN
R
The uncompensated weight is mg. Thus effective weight = VAT2 + mg = 1-56 kN acts obliquely.
1.85 Let us depict the forces acting on the small sphere m, (at an arbitrary position when the thread makes an angle 0 from the vertical) and write equation F - m vi^via projection on the unit vectors ut and un. From Ft = m wt, we have
• л
mg sin 0 - m —
vdv vdv
" m ds “ ml(-dG)
(as vertical is refrence line of angular position)
49
or
vJv- -g/sinOd0
Integrating both the sides :
V	e
fvJv = -g/f sinOdO *0	in.
or,	-y-« g I cos 0
v2
Hence — «2g cos 0 - wn	.(1)
(Eq. (1) can be easily obtained by the conservation of mechanical energy).
From F = m w Я	Л
~	n Я* V2
T — m g cos 0 = —j—.
Using (1) we have
T = 3 mg cos 0	(2)
Again from the Eq. Ft « m wt:
mg sin 0 = m wt or w; = g sin 0	(3)
Hence w - Vw2 + w* * V( g sin 0 )2 + (2g cos 0 )2 (using 1 and 3 )
 g Vl + 3 cos 2 0
(b) Vertical component of velocity, vy « v sin 0
So,	vy = v2 sin 2 0 - 2g I cos 0 sin 2 0 (using 1)
For maximum
2 d(cos0sin20) л vy or Vy , —--—-----“-0
d9
which yields
cos 0 =
1
V3
Therefore from (2) T = 3mg = V3 mg
(c) We have w= wtut + wnun thus wy -	+ wrt(y)
But in accordance with the problem wy « 0
So>	wKy) + ^(y)e 0
or,	g sin 0 sin 0 + 2g cos 2 0 (- cos 0) = 0
or,	cos 0  or, 0  54-7°
60
1.86
The ball has only normal acceleration at the lowest position and only tangential acceleration at any of the extreme position. Let v be the speed of the ball at its lowest position and I be the length of the thread, then according to the problem j- gsincx (1) where a is the maximum deflection angle
From Newton’s law in projection form : Ft« mwt
. Q dv
- mg sin 0-
or, - g I sin 0 d 0 == v dv
On integrating both lhe sides within their limits, a	0
vdv
1.87
7^//////////Л

v
о
or, v2 - 2gl (1 - cos a)	(2)
Note : Eq. (2) can easily be obtained by the conservation of mechanical energy of the ball in the uniform field of gravity.
From Eqs. (1) and (2) with 0 = a
2g/ (1 - cos a)= Ig cos a
2 or,	cos a = ~ so, a « 53
Let us depict the forces acting on the body A* (which are the force of gravity mg*and the normal Reaction N ) and write equation F« mw via projection on the unit vectors «, and u„ (Fig.)
From F « mw.
. n dv
mg sin 0» m— vdv	vdv
- m -~r~ « m ~ x ds	RdG
or, gFsin0J0 = vdv
Integrating both side for obtaining v (0)
or,
From F„ = mw.
At the moment the body loses contact with the surface, N - 0 and therefore the Eq. (2) becomes
(3)
v2  gR cos 0
51
where v and 0 correspond to the moment when the body loses contact with the surface. Solving Eqs. (1) and (3) we obtain cos 0 « ~ or, 0 * cos”1 (2/3) and v » V 2gR/3 .
1.88 At first draw the free body diagram of the device as, shown. The forces, acting on the sleeve are it’s weight, acting vertically downward, spring force, along the length of the spring and normal reaction by the rod, perpendicular to its length.
Let F be the spring force, and AZ be the elongation.
From, Fn - mwn :
Nsin 0 + F cos 0 = m co2 r
where r cos 0 « (Zo + AZ). Similarly from Ft = mwt
N cos 0 - F sin 0 « 0 or, N = F sin 0/cos 0
(1)
(2)
From (1) and (2)
F (sin 0/cos 0) • sin 0 + F cos 0 « m <o2 r
= mm2 (Zo + AZ)/cos 0
On putting F = к A Z,
к AI sin2 0 + к A Z cos2 0 « m <o2 (Zo + A I)
on solving, we get,
a f 2	zo
AZ= mco ----------z= -------z-----
к - m о) (к/m co - 1)
and it is independent of the direction of rotation.
1.89 According to the question, the cyclist moves along the circular
force is provided by the frictional force. Thus from the equation Fn = m wn
path and the centripetal
or
_______2 r Ш V . /r= -y- or kmg =
_ .'2-m v
r
ог y2= *о(г-г2/л)§
(1)
For we should have
0
or,	1 - — « 0, so r « R/2
R
HenC9 Vmax,= kogR
1.90 As initial velocity is zero thus
v2 « 2wts	(1)
As wt > 0 the speed of the car increases with time or distance. Till the moment, sliding starts, the static friction provides the required centripetal acceleration to the car.
Thus	fr« mw, but frz kmg
52
So,	w2si2g2 or, +
or,	v2*
Hence	- V Q?^-ytyR
so, from Eqn. (1), the sought distance s - ;25J£ • “d T -1 * 60 m.
zwr 2 \wt I
1.91 Since the car follows a curve, so the maximum velocity at which it can ride without sliding at the point of minimum radius of curvature is the sought velocity and obviously in this case the static friction between the car and the road is limiting.
Hence from the equation Fn - mw
kmg * or vs kRg
so
шал	mm о
(1)
We know that, radius of curvature for a curve at any point (x, y) is given as, fl + (dy/dx)2]3/2 (d2y)/dx2
(2)
R-
For the given curve,
dx
2у -a , x
= —ysin — i? a2 «
a x\ — cos — ar a
Substituting this value in (2) we get, [1 + (a2/a2) cos2 (x/a) ]3/2 (a/a2) sin (x/a)
TT .u • •	D x 71
For the minimum R, — - — a 2
and therefore, corresponding radius of curvature
Hence from (1) and (2)
vmax- a'J kg/a
1.92 The sought tensile stress acts on each element of the chain. Hence divide the chain into small, similar elements so that each element may be assumed as a particle. We consider one such element of mass dm, which subtends angle d a at the centre. The chain moves along a circle of known radius R with a known angular speed co and certain forces act on it We have to find one of these forces.
From Newton’s second law in projection form, Fx = mwx We get
2 T sin (da/2) - dN cos 0 « dm со2 Я
and from Fz - mwz we get
dVsinO « gdm
Then putting dm = mda/2 л and sin (rfa/2)« Лх/2 and solving, we get, т m + g cot 0) 2л
S3

1.93 Let, us consider a small element of the thread and draw free body diagram for this element, (a) Applying Newton’s second law of motion in projection form, Fn - mwn for this element, (T+dT)sin(dQ/2) + Tsm(dQ/2)-dN- dmu>2R- 0
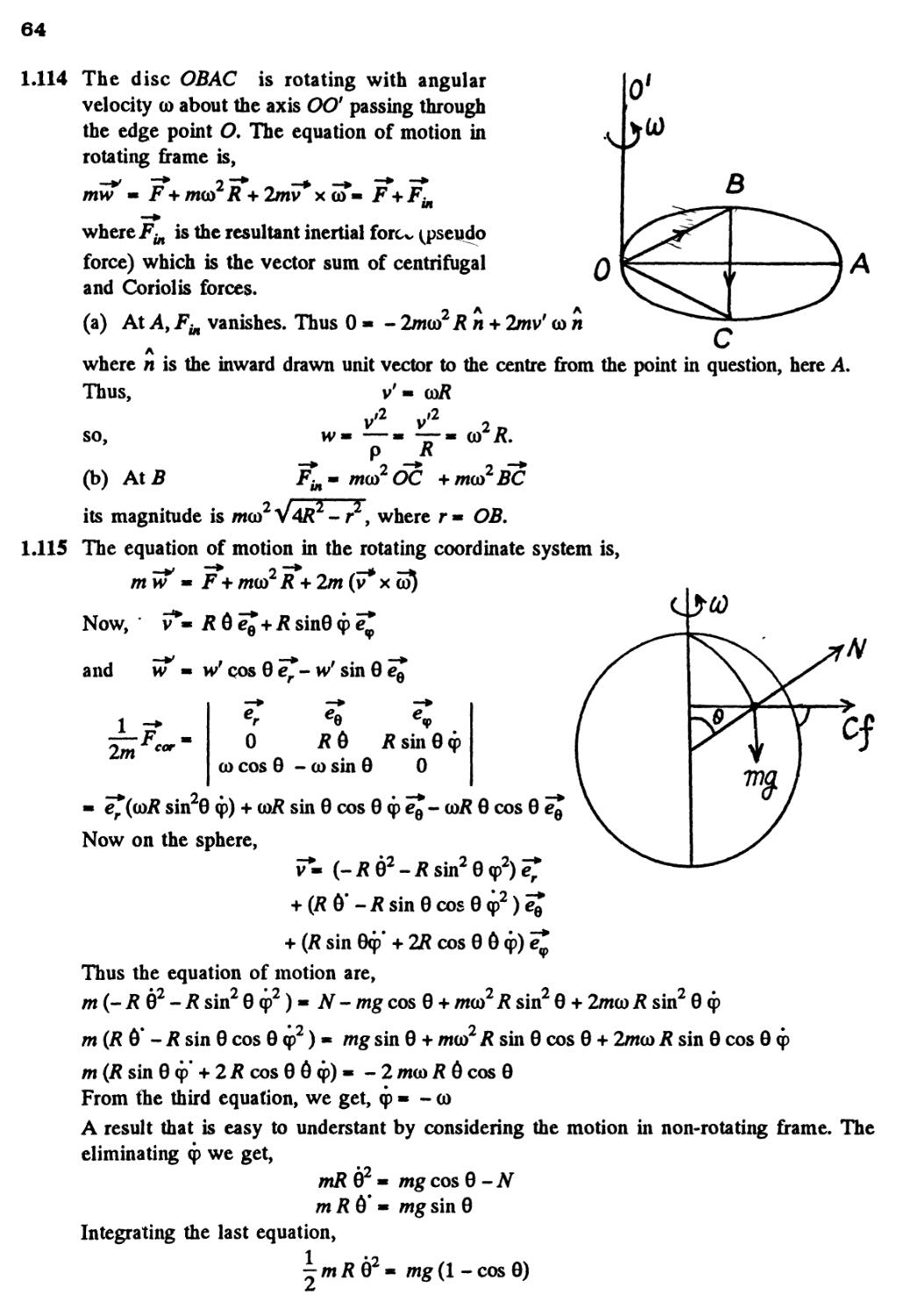
or, 2T sin (d 0/2) - dN, [negelecting the term(dT sin d 0/2) ]
or,	Td0- dN, as sin^- —	(1)
m2
(b) When — « T), \vhich is greater than the blocks will move with same value of
acceleration, (say w) and clearly m2 moves downward. From Newton’s second law in projection form (downward for m2 and upward for тг) we get :
m2S-T2~ m2w	(4)
and	~ g - m1w	(5)
64
Т2
Also	= По
21
Simultaneous solution of Eqs. (4), (5) and (6) yields :
(тг-По»1!)# (П-По) ( m2 'I
("»2 + По mi) frl + По) I mi I
1.94	The force with which the cylinder wall acts on the particle will provide centripetal force necessary for the motion of the particle, and since there is no acceleration acting in the horizontal direction, horizontal component of the velocity will remain constant througout the motion.
So	vx * v0 cos a
Using, Fn « m wn, for the particle of mass m,
2	2	2
m v* mvn cos a N = —-= —2-------------,
R A
which is the required normal force.
1.95	Obviously the radius vector describing the position of the particle relative to the origin of coordinate is
—> x —> —> —>
r = xi + у j = a sin co ti + b cos co t j
Differentiating twice with respect the time : б/ 7*	2	2
W =	= - CO (л sin О) r i + & COS О) Г / ) = - 0) r	(1)
Thus	F- mvv= -тю2г
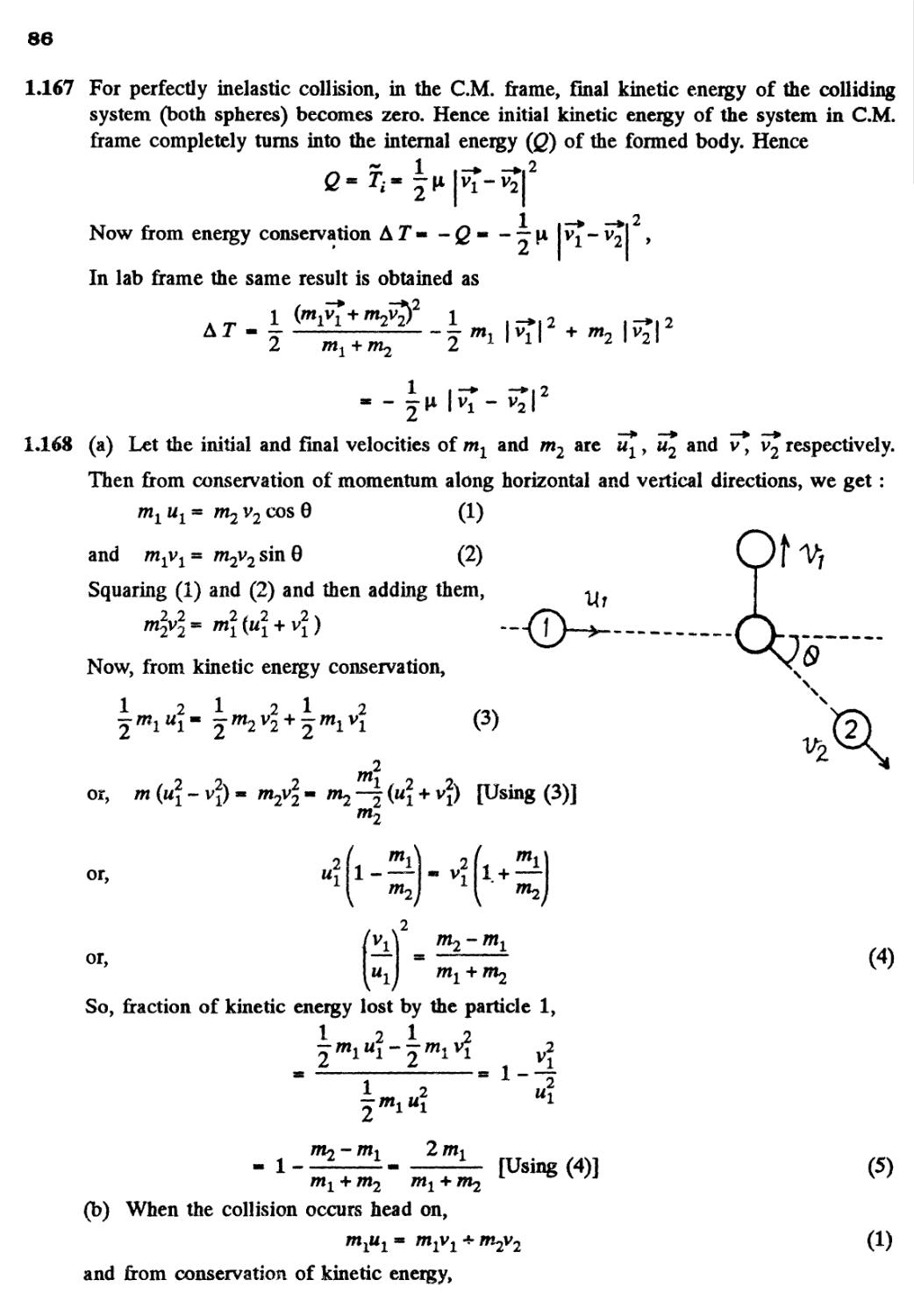
1.96	(a) We have A J* F dt t
x= Jmgiit» mgt	(1)
0 ->->
(b) Using the solution of problem 1.28 (b), the total time of motion, т =-
g
Hence using	t = т in (1)
|ДЛ
= -2m(v0-g)/g (v^-g*is-ve) ——> —
1.97 From the equation of the given time dependence force F= а Г (т - r) at f = t, the force vanishes,
(a)	Thus
о
65
or,	р - I at(x-t)dt -г-
J	6
0
but	p = mv so v- ——
6m
(b)	Again from the equation F - m w
dv*
at(x -t)~m — dt
or,	fl*(tT-r2)dt = mdv*
Integrating within the limits for v\t),
t	V*
J* a*(tx -12) dt = mJ* dv^ о	0
Hence distance covered during the time interval t  t,
5 = J* vdt 0
/at2 /т t \ , ат4 ------- I — — — I dt --
m I 2 3 m 12 0	'	7
1.98	We have F « Fo sin cot d v* —*	—> —*
or	m — “ Fo sin ua or m d v - Fo sin bitdt
On integrating, —►
-»	~F0
mv « cos <or + C, (where C is integration constant)
^0
When	t» 0, v » 0, so C » ----
mco
—>	—>
-^0	Л)
Hence, v = -----cos cor +----
m co	m co
.	Fo
As cos cot £ 1 so, v = ------(1 - cos cot)
’	mco
56
Thus	s» J* vdt
о
Fot F0sinorf Fo . « -----------x—» ------z ( СОГ - SHI (Dt )
WCO wa)2 W(l)2>
(Figure in the answer sheet).
1.99	According to the problem, the force acting on the particle of mass m is, F - Fo cos cot —►
d v* 7?	,-*^o	,
So,	m —r~ “ Fn cos cor or a v = — cos cor dt
’	dt 0	m
Integrating, within	the limits.
~	'
C J —*	C J "* Fq .
I d v = — I cos cor dt or v = ---sm cor
J	mJ	m(D
о	0
It is clear from equation (1), that after starting at t - 0, the particle comes to rest fro the first time at t« —. co —*	Л)	JC
From Eq. (1), Ivl- --------sin cor for r^ —	(2)
v 7	11 mco	co
Thus during the time interval r = л/со, the sought distance я/ш F° C •	227
---- I sincerer» 	7 mw J	m(d2
о
From Eq. (1)		
1.100 (a) From the problem		'max" — aS 1 SU1“' 1 s 1 —*	—*	d v*	—♦ F * - rv so m — » - rv dt
Thus		m — ” -rv [as d v f j v ] dt
or,		^--±dt v	m
On integrating		In v = - — t + C m
But at		Г » 0, v « v0, so, C = In v0
or,	In	V	Г — =	-•— r	or, V=	Vne	m vo	m	u
Thus for		r -♦ OOy V an 0
(b) We have m	dv dt~ “	rv so dv» -1-ds m
В7
Integrating within the given limits to obtain v (s): V	J
/J Г CJ	rs
a v  - - I as or v - v0 - — mJ	u m
vo	0
m vn
Thus for	v  0, 5  s^ - ——
(1)
r ,	mdv	dv -r ,
Let we have ---------- - r v or — - —dt
v	v m
t
or,
So
- m In (1/r)) mint)
Now, average velocity over this time interval, — InT)
Jrt
Vtf" m dt J 0___________ vo(П - 1)
<v>'p. ' =ton ‘ ЧИ
1.101	According to the problem
d v f 2 dv . л
-*v or, m—7z~-kdt dt	v2
Integrating, withing the limits,
-_f dt
V2 4
or,
m (vp-v)
* vov
(1)
To finu the valufc of ky rewrite
dv ,2 mv — » -kv
ds
or,
dv v
к , - —ds
m
On integrating
So,
. m.vQ к» ~ln~ h v
Putting the value of к from (2) in (1), we get
A(v0-v) t= --------
1 v0 vovln7
58
1.102	From Newton’s second law for the bar in projection from, Fx=* mwx along x direction
we get
or,
or,
mg sin a - fang cos a - mw
& S*n a ” aX C°S a’ (aS
v Jv « (g sin a - ax g cos a) dx
or,
vdv* gJ* (sin a -x cos a ) dx о	0
g ( sin ax - — a cos a )
2 V
2
From (1) v = 0 at either
2 x = 0, or x = — tan a
a
As the motion of the bar is unidirectional it stops after going through a distance of 2 — tan a. a
From (1), for vmax, d	2
— (sin ax - — a cos a ) » 0, which yields dx	2
So,
(1)
1, x - —tan a
a
Hence, the maximum velocity will be at the distance, x « tan a/a
Putting this value of x in (1) the maximum velocity,
Vg sin a tan a —'	a
1.103	Since, the applied force is proportional to the time and the frictional force also exists, the motion does not start just after applying the force. The body starts its motion when F equals the limiting friction.
Let the motion start after time r0 , then
F = at0 = fang or, tQ =
So, for t = £ the body remains at rest and for t > tQ obviously
= a (t - r0) or, mdv* a (t - tQ) dt
Integrating, and noting v = 0 at r « r0 , we have for t > t0 t
mdv = a I (t -10) dt or v = (t -10)2 J	M
‘o t
Thus	s= f vJr= f(r-t0)2Jr- ^-(r-r0)3
J	ш j	bm
‘o
89
1.104 While going upward, from Newton’s second law in vertical direction : vdv /	. 2ч vrfr .
m -(mg + kv) or -----------z-r- -ds
as	I	kv1}
g +--
I	mJ
At the maximum height h, the speed v = 0, so 0	h
/vdv	fjs
g + (kv2/m) J v0	0
Integrating and solving, we get,
tn	(	kv2
Л- — In 1 +-------
2k	\	mg
When the body falls downward, the net force acting on the body in downward direction equals (mg-kv2),
Hence net acceleration, in downward direction, according to second law of motion vdv kv2 ds % m
(1)
vdv kv2
m
h
or,
Thus
vdv Г , ------z—= I ds g-kv/m J о °---о
Integrating and putting the value of h from (1), we get,
v'- v0/ >/ l + k v$/mg.
1.105	Let us fix x - у co-ordinate system to the given plane, taking x-axis in the direction along which the force vector was oriented at the moment t- 0, then the fundamental equation of dynamics expressed via the projection on x and у-axes gives, d v
Feos cor-	(1)
and
dv dt
(a) Using the condition v(0) « 0, we obtain vx -
F .
---sm co t m co
and
v в ----(1 - cos co t)
y mco
(2)
(3)
(4)
Hence,
60
(b) It is seen from this that the velocity v turns into zero after the time interval Ar, which can be found from the relation,
Ar ~	,
co —= л. Consequentely,
the sought distance, is
ДГ
Average velocity, < v > «
Svdt
mco2 о
2 л/®
So, <v> = I
0
4F лт(о
1.106	The acceleration of the disc along the plane is determined by the projection of the force of gravity on this plane Fx « mg sin a and the friction force fr - kmg cos a. In our case
к = tan a and therefore
fr» Fx»mgs\TLV.
Let us find the projection of the acceleration on the derection of the tangent to the trajectory and on the x-axis :
m wt» Fx cos qp - fr = mg sin a ( cos qp - 1 )
mwx» Fx- fr cos qp « mg sin a (1- cos qp ) It is seen fromthis that « - wx, which means that the velocity v and its projection vx differ only by a constant value C which does not change with time, i.e.
v» vx + C,
where vx » v cos qp. The constant C is found from the initial condition v» v0, whence
it
C » v0 since qp - — initially. Finally we obtain
v = v0 / (1 + cos qp).
In the cource of time qp 0 and v -* Vq/2. (Motion then is unaccelerated.)
1.107	Let us consider an element of length ds at an angle qp from the vertical diameter. As the speed of this element is zero at initial instant of time, it’s centripetal acceleration is zero, and hence, d#-Xdfccosqp = 0, where X is the linear mass density of the chain Let T and T+dT be the tension at the upper and the lower ends of ds. we have from, Ft = mwt
(T+ dTj + Xdsgsinqp-Ts Mswt
or,	dT + к	qp g sin qp = Xds wt
61
If we sum the above equation for all elements, the term j* dT = 0 because there is no tension at the free ends, so l/R
j sin ф d ф « J ds » XI wt о

gR /	I
Hence w(= ,	1 - cos ~
/ |	R
As wn = a at initial moment
So, w =
1.108	In the problem, we require the velocity of the body, realtive to the sphere, which itself moves with an acceleration w0 in horizontal direction (say towards left). Hence it is advisible to solve the problem in the frame of sphere (non-inertial frame).
At an arbitary moment, when the body is at an angle 0 with the vertical, we sketch the force diagram for the body and write the second law of motion in projection form F„ = mw n n
________________________ 2 л жг	• Л ft^V
or, mg cos 0 - N - mwQ sin 0 =
At the break off point, N = 0, 0 = v= v0so the Eq. (1) becomes,
V°2	0	-0
-Д- - g cos 0O - w0 sin 0O
From, Ft« mwt v dv mg sin 0 - mw. cos 0 = m -r- « m _ , _ °	u	ds RdQ
or, v dv = R (g sin 0 + w0 cos 0 ) d 0
vo	e0
(1)
and let
vdv
(2)
%
о
(3)
I R (g sin0 + w0 cos0) d 0 о v02 — - g(l - COS0O) + H>0 sin 0O
Note that the Eq. (3) can also be obtained by the work-energy theorem A = A T (in the frame of sphere)
1	2
therefore, mgR (1 - cos 0O) + mwQ R sin 0O » — mvQ [here mwQR sin 0O is the work done by the pseudoforce (- mwo)]
Vo2
— = g (1 - cos 0O) + w0 sin 0O
Zj\
or,
62
Solving Eqs. (2) and (3) we get,
~	. n < Г 2 + ky/5 + 9k2
v0 - V2&R/3 and 0n»cos 1 -----5--
°	I 3(1+P)
w0 , where к » — g
Hence
17°
1.109
This is not central force problem unless the path is a circle about the said point. Rather here Ft (tangential force) vanishes. Thus equation of motion becomes, vf« v0« constant __________________________________2 mv0
and,
A F — for ro
We can consider the latter equation as the equilibrium under two forces. When the motion is perturbed, we write r
mvp _ r0+x“
r0 + x and the net force acting on the particle is, ,	.	2 , k	2
А Г nx\	x \ m v0
“ 1-— +------- 1-— »-—т-(1-л)х
“I ro ro I ro r*
(mv mVo —— is
-A
force while is thd inward directed external force).
an outward directed centrifiigul
1.110
There are two forces on the sleeve, the weight Fr and the centrifugal force F2. We resolve both forces into tangential and normal component then the net downward tangential force on the sleeve is, . _Л <о2л mg sm0 11 - - - cos 01
This vanishes for 0 = Oand for 0 = 0O « cos ”1
L which is real if
rntf-RSindCosO
mjFRSinQzFz
f	2
co2 R > g. If co2 R < g, then 1 - — -- cos 0 g
is always positive for small values of 0 and hence the net tangential force near 0« 0 opposes any displacement away from it. 0 = 0 is then stable.
2 R If co2 R > g, 1-----cosO is negative for small
0 near 0=0 and 0 » 0 is then unstable.
However 0 « 0O is stable because the force tends to bring the sleeve near the equilibrium position 0  00-
If co2 Я = g, the two positions coincide and becomes a stable equilibrium point.
'	^Cosd+TntfLRSuP
A
63
1.111
Define the axes as shown with z along the local vertical, x due east and у due north. (We assume we are in the northern hemisphere). Then the Coriolis force has the components.
Fcor = -2m(saxv}
- 2mco £ vy cosG - v2 sinG) i - vx cosG j + vx cosG к 1» 2m<o (vy cosG - vz sinG) i since vxLis small when the direction in which the gun is fired is due north. Thus the equation of motion (neglecting centrifugal forces) are x « 2mco (yy sinqp - vz coscp), у « 0 and z » - g Integrating we get у • v (constant), z = - gt and i - 2co v simp t + cog t2 cosqp Finally, 2 .	1	3
x= covr sinqp + jcogt cosqp
Now v » gt in the present case, so, /s\2	s2
x* covsin<p|~l = CDsinqp —
(О
О=90° У
1.112
1.113
* 7 cm (to the east).
Z-y/ertaxd
5c-£^sC
The disc exerts three forces which arc mutually perpendicular. They are the reaction of the weight, mg, vertically upward, the Coriolis force 2mv' co perpendicular to the plane of the vertical and along the diameter, and mco2r outward along the diameter. The resultant force is,
F » m V g2 + w4 r2 + (2v' co)2
The sleeve is free to slide along the rod AB. Thus only the centrifugal force acts on it. The equation is,
mv-
mco2r
1- dr where v « — • dt
But
dv
V dr =
d 11 2 dr 2V

so,
or,
1	2 2
— cd r + constant
2
2	2	2 2
V - vo + to r
v0 being the initial velocity when r = 0. The Coriolis force is then,
2/HCo Vvq + co2 r2 = 2?nco2 r Vl + Vq/co2 r2
= 2-83 N on putting the values.
64
1.114
The disc OBAC is rotating with angular velocity co about the axis OO' passing through the edge point O. The equation of motion in rotating frame is,
mw « F + mupR + 2mv* x ST- F + F^
where F^ is the resultant inertial forcv (pseudo force) which is the vector sum of centrifugal and Coriolis forces.
(a)	At A, Fj* vanishes. Thus 0  - 2mu>2 Rn + 2mv' co n
where n is the inward drawn unit vector to the centre from the point in question, here A.
Thus,	v'- соЯ
v'2 va 2 D so,	w- —ж — » (0 R.
— p
(b)	At В	F^- ты2 ОС + mw2BC
its magnitude is mco2 V1R2 - r2, where r » OB.
1.115
The equation of motion in the rotating coordinate system is,
/ий^ж Г + /исо2Я + 2/л(у*xco)
Now, ' v*- R 0 +R sin0 cp ej
and w • w' cos 0 er - wf sin 0 e0
e	€
г	О	Ф
О Я 0 Я sin 0 cp co cos 0 - co sin 0	0
• е^(соЯ sin20 cp) + соЯ sin 0 cos 0 ср соЯ 0 cos 0 Now on the sphere,
v*- (- Я 02 - Я sin2 0 ф2)
+ (Я 0’ - Я sin 0 cos 0 ф2) ej
+ (Я sin 0cp’ + 2Я cos 0 0 ср) e*
Thus the equation of motion are, m (- R 02 - Я sin2 0 ф2) » N - mg cos 0 + /исо2Я sin2 0 + 2mco Я sin2 0 cp
m (R 0 - Я sin 0 cos 0 ф2)« mg sin 0 + /то2 Я sin 0 cos 0 + 2/исоЯ sin 0 cos 0 cp
m (R sin 0 ср + 2Я cos 0 0 cp) - - 2 /исо Я 0 cos 0
From the third equation, we get, cp » - co
A result that is easy to understant by considering the motion in non-rotating frame. The eliminating cp we get,
mR 02 » mg cos Q -N
mR& » mg sin 0
Integrating the last equation, 1 -o - w Я 0 » mg (1 - cos 0)
65
Hence	N « (3-2 cos 0) mg
_ i 2
So the body must fly off for 0 = 0O « cos “	exactly as if the sphere were nonrotating.
2	/5	2
Now, at this point FCf « centrifugal force « mco R sin 90 « у — mco R
Fear - V co2 R2 02 cos2 0 + (co2 Л2)2 sin2 0 x 2m
- д/ (<o2 Л )2 + co2 Л2 x x x 2m - |mo)2 R д/б +——
v 9	9 3R 3 v 3u>2R
1.116 (a) When the train is moving along a meridian only the Coriolis force has a lateral component and its magnitude (see the previous problem) is,
2m co v cos 0 - 2m w sin X
(Here we have put Л Й-* v)
, - 2 X 2000 X 103 X -^-X lateral	86400
« 3-77 kN, (we write Xfor
(b) The resultant of the inertial forces acting on the train is, M
F:-- 2mtnR 0 cos 0 e*
+ (m<$2R sin 0 cos 0 + 2m wR sin 0 cos 0 <p)
+ (mco2 R sin2 0 + 2m co R sin2 0 ср) ё*
So,
x
3600	2
the latitude)
This vanishes if 6=0, <p«-^co
v*= vm = -—cousin©» -—coKcosX ф ф ’ <p 2	2
(We write X for the latitude here)
Thus the train must move from the east to west along the 60th parallel with a speed, |<o^cosk- 4x7^7X 1O‘4X6-37x 106- 115-8 m/s-417 km/hr 2	4 8-64
1.117 We go to the equation given in 1.111. Here vy = 0 so we can take у = 0, thus we get for the motion in the x z plane.
x = - 2co vz cos 0
z ж -g
Z--|g*2
*	2
x = cog cos cpf
t v3/2
1	.3 1	/2Л\
— (О g cos <p t « — co g cos <pl —
3	3	I g j
2&h
з v g
There is thus a displacement to the east of
2 2n^ cnn л -/400	_
— x — 64 x 500 x 1 x у -т-у - 26 cm.
3 о	’ У’о
Thus
and
Integrating,
So
66
1.3 Laws of Conservation of Energy, Momentum and Angular Momentum.
1.118 As F is constant so the sought work done
A - FAF*- F- (^- rf)
or, A- (3 i+4j*) • [ (2 Г- ЗЛ - (i + 2j*) ] - (3 i+ 4/*) • (T-sf) - 17 J 1.119 Differentating v (s) with respect to time
dv a ds a	a2
dt 2 vs di 2 vs	2
(As locomotive is in unidrectional motion) ma2 Hence force acting on the locomotive F • m w - ——
Let, at v - 0 at t - 0 then the distance covered during the first t seconds 1	1	л2	„2
1 .2	1	.2	в .2
5 = — Wt = —-s-Г = — t
2	2	2	4
tt .u	v	i	a	₽ тд2	(tf2/2)
Hence the sought work, A = Fs = —---------
mat2 8
1.120 We have
T 1	2	2	2 2 as2
T« ~mv « as or, v - ----------
2	m
Differentating Eq. (1) with respect to time
(1)
(2)
Hence net acceleration of the particle
7^74-. V(—— Vi.(W
1 n ¥ I m I I mR j m
Hence the sought force, F = mw = 2as Vl + (y/K)2
—>
1.121	Let F makes an angle 0 with the horizontal at any instant of time (Fig.). Newton’s second law in projection form along the direction of the force, gives :
F = kmg cos 0 + mg sin 0 (because there is no acceleration of the body.)
As F f f d r*the differential work done by the force F, dA = F -dr= Fds, (where ds 
« kmg ds (cos 0) + mg ds sin 0 = kmgdx + mgdy.
I	h
Hence, A - kmg I dr + mg I dy о	0
= kmgl + mgh= mg(kl + h).
N
fr
>
67
1.122 Let s be the distance covered by the disc along the incline, from the Eq. of increment of M.E. of the disc in the field of gravity : AT + At/  A, 0 + (- mgs sin a)« - kmg cos a 5 - kmg I
kl
or,
(1)
5
sin a - к cos a Hence the sought work Ajr « - kmg [5 cos a + I ] Af, “ ~ 1-^fcofta [Using the Eqn’ (1)1
On puting the values « -0.05 J
1.123 Let x be the compression in the spring when the bar m2 is about to shift Therefore at this moment spring force on m2 is equal to the limiting friction between the bar m2 and horizontal floor. Hence
кx « km2g [where к is the spring constant (say)]	(1)
For the block mx from work-energy theorem : A  AT « 0 for minimum force. (A here indudes the work done in stretching the spring.) 1	2	X
so,	Fx- — Kx-kmgx^ 0 or K~-F-hn1g
From (1) and (2),
Hl
) fa
(2).

F~ kg
1.124	From the initial condition of the problem the limiting fricition between the chain lying on the horizontal table equals the weight of the over hanging part of the chain, i.e.
X T] Ig « к к (1 - T]) Ig (where X is the linear mass density of the chain)
1-n
Let (at an aibitrary moment of time) the length J 1 * * of the chain on the table is x. So the net friction force between the chain and the table, at this moment : fr- kN~ kkxg	(2)
The differential work done by the friction forces :
dA« fr-dr*- -frds - -kKxg(-dx)-
(Note that here we have written ds • - dx., because ds is essentially a positive term and as the length of the chain decreases with time, dx is negative)
Hence, the sought work done о
N
So,


(1)
X

(3)
A-
-1’3 J
(1-nH
68
1.125	The velocity of the body, t seconds after the begining of the motion becomes vj + g*i. Thb power developed by the gravity (m g*) at that moment, is
p« mg*-v*« m(g*- Vo + g2O“ mg (gt - v0 sin a)	(1)
As mg* is a constant force, so the average power
<р>_Аж mglAF* T T
where Дг* is the net displacement of the body during time of flight
As,	mg*± Ar* so <P> « 0
V2 2	r—
1.126	We have	wn - —« at , or, v - yaR t,
R
t is defined to start from the begining of motion from rest
So,	w «	= VaR"
-> dt
Instantaneous power, P = F • v*= m (wt ut +	ut ) • (т/aR t ut ),
(where ut and ut are unit vectors along the direction of tangent (velocity) and normal respectively)
So,	P- mwt4aRt^ maRt
Hence the sought average power t	t
J* Pdt J* maRtdt
<P>-±---------------------
'	t
J dt о
Hence
maRt2 ma Rt
It “	2
1.127
Let the body m acquire the horizontal velocity v0 along positive x - axis at the point O.
(a) Velocity of the body t seconds after the begining of the motion, V- Vo + wr- (vQ-kgt)i*	(1)
—>	—♦
Instantaneous power P - F • v - (- kmg i ) • (v0 - kg t) - kmg (v0 -kgt)
From Eq. (1), the time of motion т » v</kg
Hence sought average power during the time of motion T
J* - kmg (v0 - kgt) dt
<P> - ---------------------- - —• - 2 W (On substitution)
X	Xr
From/7* - mwx
dvx
-kmg= mwx^ mvx —
or,	vxdvx~ -kgdx « - OLgxdx
69
To find v (x), let us integrate the above equation V	X
J* vxdvx- - agj*xdx or, v2- vfi-agx?	(1)
vo	0
Now,	P = F- v*= -maxgyift- agx2	(2)
For maximum power, (Vv20x2 -kgx4) « 0 which yields x -
Putting this value of x, in Eq. (2) we get,
pm«- - ^mvlVag
1.128 Centrifugal force of inertia is directed outward along radial line, thus the sought work r2
A- j* m(°2 r^ras 2 m(°2 (r% ~ s 0’20 T substitution) ri
1.129 Since the springs are connected in series, the combination may be treated as a single spring of spring constant
к- K1*2
K1 + K2
From the equation of increment of M.E., Д T + Д U •
1	9	KK2 \	.2
О + ^кД/М, or,	—— Д/2
2	2 kx + k2 j
1.130 First, let us find the total height of ascent At the beginning and the end of the path of velocity of the body is equal to zero, and therefore the increment of the kinetic energy of the body is also equal to zero. On the other hand, in according with work-energy theorem ДТ is equal to the algebraic sum of the works A performed by all the forces, i.e. by the force F and gravity, over this path. However, since AT» 0 then A = 0. Taking into account that the upward direction is assumed to coincide with the positive direction of the у - axis, we can write h	h
A = (f +	r*= §(Fy-mg)dy
о	о
h
« m&J* (1 ” аУ) ж mS^ (1 atys 0. о whence h - 1/a.
The work performed by the force F over the first half of the ascent is h/2	h/2
AF- J* Fydy~ 2mg J*(1 - ay) dy - 3 mg/4a. о	о
The corresponding increment of the potential energy is
Д U = mgh/2 = mg/2a.
70
1.131
From the equation Fr~ - “7 we get Ft
a b
3 + 2
(a) we have at r - r0, the particle is in equilibrium position. i.e. Fr « 0 so, r0 «
To check, whether the position is steady (the position of stable equilibrium), we have to satisfy
d 2U n ---5">° dr2
d2U dr2 2a Putting the value of r « r0 - —, we get
We have
6a 2b
4 “ 3
So,
U b4
~ ’ (3S a аП<1 аГС Pos^e constant)
d2U b2 n -----ж —->0, dr2 8a3
which indicates that the potential energy of the system is minimum, hence this position is steady.
(b) We have
dU dr
dFr
For F, to be maximum,	—г~ ж 0
dr
3a	b3
So, r - — and then - ---------тг,
b	r(max) 27a2
2a b
As Fr is negative, the force is attractive.
1.132 (a) We have
Fx- -2ax and F -	-2$y
x dx	y dy
So,	F*- 2axF^2Py Tand, F- 2V а2x2 + p2y2	(1)
For a central force, r x F - 0 —> —>	—► —>	—>	—♦
Here,	rxF* (xi+yj )x(-2axi-2Pyj )
= - 2fixy k-2axy (к )и 0
Hence the force is not a central force.
(b) As ar2 + py2
So’ Fx° ^дх° ~2ax and Fy~ “д)Г“ -20у-
So,	F- +	» V 4а2^ + 4^2у2
According to the problem
F » 2 V a2 x2 + p2 y2 » C (constant)
71
or,

?	/ c2 ,
or,	-^• + '4-- —r-r- A: (say)
p2 a2	2a2p2	”
Therefore the surfaces for which F is constant is an ellipse.
For an equipotential surface U is constant
So,	a x2 + p y2 - Co (constant)
(2)
x2	y2	co
or,	-= + -j= - — - Ko (constant)
V p2	Va2	a ₽
Hence the equipotential surface is also an ellipse.
1133 Let us calculate the work performed by the forces of each field over the path from a certain point 1 (xt, уг) to another certain point 2 (x2, y2)
X2
(i) dA~ F' dr^ ay i-dr^ aydx or, A - a J* ydx
(ii) dA * F 'dr*» (axi + byi ) • dr*~ axdx + bydy
X2	У2
Hence	A- J* a xdx + bydy
XL	У1
In the first case, the integral depends on the function of type у (x), i.e. on the shape of the path. Consequently, the first field of force is not potential. In the second case, both the integrals do not depend on the shape of the path. They are defined only by the coordinate of the initial and final points of the path, therefore the second field of force is potential.
1.134	Let be the sought distance, then from the equation of increment of M.E. AT+ACJ- Afr
^0-^mvj) j + (+?ng s sina) «= -fangcosas
v2/
or,	5 - — / (sin a + к cos a)
- ктУ^
Hence	- kmg cos a s - 777;---7
6	2 (k + tan a)
1.135	Velocity of the body at height A, vh = V 2g (H - h), horizontally (from the figure given in the problem). Time taken in falling through the distance h.
Now

(as initial vertical component of the velocity is zero.)
s= vht= y/2g(H + h)	y/4(Hh-h1}
s
72
For 5^, -у- (Hh - ti2)  0, which yields h « ~ US	£
Putting this value of h in the expression obtained for s, we get, Smax= H
1.136
To complete a smooth vertical track of radius R, the minimum height at which a particle starts, must be equal to (one can proved it from energy conservation). Thus in our
problem body could not reach the upper most point of the vertical track of radius R/2,
Let the particle A leave the track at some point О with speed v (Fig.). Now from energy conservation for the body A in the field of gravity :
mg
h - (1 + sin 0)
-imp2
or, v2« gh (1 - sin 0)	(1)
From Newton’s second law for the particle at the point O; Fn = mwn,
»jr	• л 7WV
JV.mSsmS-
But, at the point О the normal reaction jV - 0
So, v2-^sin0	(2)
From (3) and (4), sin 0 =
2
j and v -
After leaving the track at O9 the particle A comes in air and further goes up and at maximum height of it’s trajectory in air, it’s velocity (say v') becomes horizontal (Fig.). Hence, the sought velocity of A at this point
v'» v cos (90 - 0) = v sin 0
1.137	Let, the point of suspension be shifted with velocity vA in the horizontal direction towards left then in the rest frame of point of suspension the ball starts with same velocity horizontally towards right Let us work in this, frame. From Newton’s second law in projection form towards the point of suspension at the upper most point (say B) :
2	2
mvo	mv»
mg + T- or, T- —-mg	(1)
Condition required, to complete the vertical circle is that T 0. But	(2)
|mv2- mg(2/) + |mv2 So, vjj- v^-4g/	(3)
73
From (1), (2) and (3)
m (va “ 4 8l)	.___
T _ ---------_ mg г о or> s y5 gi
Th»18 VA№-yfcgl
From the equation Fn • mwn at point C
r-Y	(
Again from energy conservation
|wva" ^mv2c + mgl	& VA
(5)
From (4) and (5)
T- 3mg
1.138	Since the tension is always perpendicular to the velocity vector, the work done by the tension force will be zero. Hence, according to the work energy theorem, the kinetic energy or velocity of the disc will remain constant during it’s motion. Hence, the sought time
” , where 5 is the total distance traversed by the small disc during it’s motion.
Now, at an arbitary position (Fig.) (Zo-/?O)d0,
l/R
so, s - f (Zo - К 0) d 0 о
li 1L
ОГ’	2Д2ЯВ2Я
Hence, the required time, t  у
It should be clearly understood that the only uncompensated force acting on the disc A in this case is the tension T, of the thread. It is easy to see that there is no point here, relative to which the moment of force T is invarible in the process of motion. Hence conservation of angular momentum is not applicable here.
1.139 Suppose that AZ is the elongation of the rubbier cord. Then from energy conservation, AU^ + ACZ^- 0 (as AT« 0)
or, - mg (I + AZ) + ~ к A/2 - 0
1 <>
or,	— к AZ2 - mg Al - mg I • 0
74
Mg*V (mg)2 + 4x^mgl K	/; 2К/
or. Д/» ---------------!----------i----x — = —°- 1 + v 1 ±------
9 к	2 к * mg J
Z 2
/	2к/
Since the value of *V1 +--- is certainly greater than 1, hence negative sign is avoided.
T	mg
о	л, I л - Г 2 к/ I
So,	Л/ =	+ V 1 +--
к \ v mg /
1.140 When the thread JM is burnt, obviously the speed of the bars will be equal at any instant of time until it breaks off. Let v be the speed of each block and 0 be the angle, which the elongated spring makes with the vertical at the moment, when the bar A breaks off the plane. At this stage the elongation in the spring.
Al = lQ sec 0 - lQ «lQ (sec 0-1)	(1)
Since the problem is concerned with position and there are no forces other than conservative forces, the mechanical energy of the system (both bars + spring) in the field of gravity is conserved, i.e. AT + AU « 0
1.141
So,
2 I ~ mv21 + — к /02 (sec 0 - I)2 - mglQ tan 0 « 0
From Newton’s second law in projection form along vertical direction : mg- N+kIo (sec 0-1) cos 0
But, at the moment of break off, N « 0.
Hence, к /0 (sec 0-1) cos 0 « mg
K/0-mg COS 0 = -------
kZ0
Taking к « ^-^4 simultaneous solutiorixjf (2) and (3) yields : *o
or,
(3)
(T
(2)
32
Obviously the elongation in the cord, Al» Zo (sec 0 - 1), at the moment the sliding first starts and at the moment horizontal projection of spring force equals the limiting friction.
So,	Al sin 0= kN
(where Kr is the elastic constant).
From Newton’s law in projection form along vertical direction :
or,
From (1) and (2),
Kj Al cos 0+7У® mg.
N = mg - Kj Al cos 0
Al sin 0 - к (mg - Al cos 0)
(1)
KAl
У 9
75
°Г’ K1 AZ sin 0 + к AZ cos 0
From the equation of the increment of
mechanical energy : At7 + AT« Afr
or,	^iK1A/2j-A/r
fang AZ 2	A
ОГ’ 2 Д/ (sin 0 + k cos 0) " fr
kmg L (sec 0-1)
Thus « • 77- г—i-------------0'09 J (on substitution)
fr 2 (sin 0 - A: cos 0)	v	7
1.142 Let the deformation in the spring be AZ, when the rod AB has attained the angular velocity co.
From the second law of motion in projection form Fn « mwn .
о	mo)2 Zft
к AZ = mor (lQ + AZ) or, AZ = ------2
к - тог 1	2	1	°
From the energy equation, A^ » j + у к AZ"
- |пко2(/о + А/)2 + |кД/2
2	2
. Zmrn2/2\ | I /ИО) Iq
+ -K ------J
(к - m<o у
1	26 OT“2/o '
Zo +-------j
( к - mto у
к Л О- *r.)	- men2
—----------— where n «  
2 (1-пГ	к
1.143 We know that acceleration of centre of mass of the system is given by the expression. mlwl^m2w2 -------------------------------------------------
On solving
A act
+ m2
Since
Wi « - w2
w —---------------
c m,i + m2
Now from Newton’s second law F - mw, for
the bodies mt and m2 respectively.
T + mr
	j,
and T + m2 g - m2 w2 « - m2 Wj
(1)
(2)
(3)
Solving (2) and (3) _>	(m1-m2)g
----
m1 + m2
(4)
76
Thus from (1), (2) and (4),
—	("»i - mJ2 g
Wc“ ----------2~
(Mj + M2)
1.144 As the closed system consisting two particles and of m2 is initially at rest the C.M. of the system will remain at rest. Further as m2 « m^/2, the C.M. of the system divides the line joining and m2 at all the moments of time in the ratio 1 : 2. In addition to it the total linear momentum of the system at all the times is zero. So, «= - p2 and therefore the velocities of and m2 are also directed in opposite sense. Bearing in mind all these thing, the sought trajectory is as shown in the figure.
1.145 First of all, it is clear that the chain does not move in the vertical direction during the uniform rotation. This means that the vertical component of the tension T balances gravity. As for the horizontal component of the tension Г, it is constant in magnitude and permanently directed toward the rotation axis. It follows from this that the C.M. of the chain, the point C, travels along horizontal circle of radius p (say). Therefore we have,
T cos 0 « mg and T sin 0 - mco2 p
_.	gtanO n o
Thus p « D—x— « 0-8 cm
O)
and T--^-5N cos 0
1.146
(a) Let us draw free body diagram and write Newton s second law in terms of projection horizontal direction respectively.
N cos a - mg + fr sin a « 0
(1)
fr cos a - N sin a - mat21
(2)
From (1) and (2)
fr cos a - S1U C~ (- fr sin a + mg) = zwto21
J	cos a
along vertical
and

77
So,
f r = mg I sin a +--------cos a I = 6N
к 8	/
(3)
(b) For rolling, without sliding, fr* JtN
but, N « mg cos a - pi cd 2 / sin a
mg sin a +-------cos a s; к ( mg cos a - pi cd 2 I sin a) [Using (3)]
\	8	/
Rearranging, we get, m cd 21 ( cos a + fcsina) *(k mg cos a - mg sin a )
Thus	co s V g (£ - tan a )/(1 + fctan a ) I = 2 rad/s
1.147 (a) Total kinetic energy in frame К* is
—>
This is minimum with respect to variation in V, when
0, i.e. mr ( - V)2 + m2 ( v2 “ Ю “ 0
Hence, it is the frame of C.M. in which kinetic energy of a system is minimum.
(b) Linear momentum of the particle 1 in the К' or C frame r r mr m2 T >
Л =	—-—(h-v2)
т "»2
> x	mY m2
or,	Pi = u ( v, - v2), where, p = -» reduced mass
mr + m2
Similarly,	= p(v^-v^)
So,	1л1= 1Я1= P=	where, vrd« |v^-v^|	(3)
Now the total kinetic energy of the system in the C frame is ±2	±2
т= т. + т,-	+	£-
1	2 2mr 2m2	2 p
~	1	2	1	। -♦ -* .2
Hence	T= - ц = - ц vx - v2
78
1.148 To find the relationship between the values of the mechanical eneigy of a system in the К and C reference frames, let us begin with the kinetic eneigy T of the system. The velocity of the i-th partide in the К frame may be represented as vf+ • Now we can write
° 2	+ OT«i'i + S lw»vc
Since in the C frame	0, the previous expression takes the form
1	7	1	7
T« T+ vc - T+-m V (since according to the problem vc - V)	(1)
Since the internal potential eneigy U of a system depends only on its configuration, the magnitude U is the same in all refrence frames. Adding U to the left and right hand sides of Eq. (1), we obtain the sought relationship
E- E + |m V2
1.149	As initially U= &= 0, so, E- T
From the solution of 1.147 (b)
|nlh-^b
As	v^±
Thus
1.150	Velocity of masses and m2, after t seconds are respectively. . v^ = i\ + g*r and =
Hence the final momentum of the system, p*=	=	+
- pl+mg*t, (where, - m2 + m2 and m - m1 + m2)
	- fr 1 _.fr 2
And radius vector,	rc - vc t + — wc t
+	2__2
(mj+.'nj) +2gt
1 —» 2	v _ mrf+m^
 vot + -gt , where v0--------------
u 2	u mt + m2
79
1.151 After releasing the bar 2 acquires the velocity v2, obtained by the energy, conservation : 1	2	1	- / к
or, v2.xy-	(1)
Thus the sought velocity of C.M.
0 + m2 x V m2	x Vm2K
Vcm mi + m2 (+ m2)
1.152 Let us consider both blocks and spring as the physical system. The centre of mass of the F
system moves with acceleration a « --------------towards right Let us work in the frame of
+ m2
centre of mass. As this frame is a non-inertial frame (accelerated with respect to the ground) we have to apply a pseudo force a towards left on the block zn1 and m2 a towards left on the block
iqa
As the center of mass is at rest in this frame, the blocks move in opposite directions and come to instantaneous rest at some instant. The elongation of the spring will be maximum or minimum at this instant. Assume that the block m1 is displaced by the distance xx and the block m2 through a distance x2 from the initial positions.
From the energy equation in the frame of C.M.
AT+	,
(where also includes the work done by the pseudo forces) Here,
ДТ-0, U^^k(x1+x2f and
a
or,
So.
(F-m2F\ m1F mlF(x1+x2) ' ------- x2 +------xi=----------->
m I + m2 j z m1 + m2	+ m2
1	, ,	.2 ^(x^x^F
— Jc(x, + x9) = ------
2	1	2' m1 + m2
2 mrF x0 « 0 or, x. + x? « —--г
2	1	2 к (тг + m2)
2m{F
Hence the maximum separation between the blocks equals : Zo + —---г
к (mi +
Obviously the minimum sepation corresponds to zero elongation and is equal to Zo
1.153 (a) The initial compression in the spring AZ must be such that after burning of the thread, the upper cube rises to a height that produces a tension in the spring that is atleast equal to the weight of the lower cube. Actually, the spring will first go from its compressed
80
state to its natural length and then get elongated beyond this natural length. Let I be the maximum elongation produced under these circumstances.
Then
к I - mg	(1)
Now, from energy conservation,
у к A/2-mg(A/ + /) + |K/2	(2)
(Because at maximum elongation of the spring, the speed of upper cube becomes zero) From (1) and (2),
д/2_2щ>Д/_	0	д;_ 3mg_
К к2	к к
Therefore, acceptable solution of Д/ equals
(b) Let v the velocity of upper cube at the position (say, at C ) when the low.er block breaks off the floor, then from energy conservation.
|mv2- |K(A/2-/2)-OTg(/ + A/)
(where I - mg/к and ДI - 7	)
or,
v2- 32^ к
(2)
At the position C, the velocity of C.M; vc« (spring* two cubes) further rises up to Now, from energy conservation,
l(2/n)v2- (2m)gArC2
mv + 0 v
—-----« — -Let, the C.M. of the system
2m 2
or,
ДУС2
But, uptil position C, the C.M. of the system has already elevated by,
дУс1-
(Д/ + /) m + 0	4 mg
2m	к
Hence, the net displacement of the C.M. of the system, in Upward direction aaa 8 mg дУс“ ^Ус1 + ^Ус2~
1.154 Due to ejection of mass from a moving system (which moves due to inertia) in a direction perpendicular to it, the velocity of moving system does not change. The momentum change being adjusted by the forces on the rails. Hence in our problem velocities of buggies change only due to 1he entrance of the man coming from the other buggy. From the
81
Solving (1) and (2), we get
As	vx f j v and v2 11 v
o	-► -mv* , -> Mv*
So-	(ЙГ^)
1.155 From momentum conservation, for the system “rear buggy with man”
(M + m)vo~ m(u + v^)+Mv^	(1)
From momentum conservation, for the system (front buggy + man coming from rear buggy) Mi£+m(u*+i£)« (M+m)v^
So,
V»« 77--+ 77---(M + Vi?)
p Mim M+m R
Putting the value of v£ from (1), we get
vF- v0 +
mM -> -------о w (M + m)2
1.156 (i) Let vx be the velocity of the buggy after both man jump off simultaneously. For the closed system (two men + buggy), from the conservation of linear momentum, Mv^+2m(u +v^)- 0
or,
->	-2ma*
V1“ M+2m
(1)
(ii) Let v* be the velocity of buggy with man, when one man jump off the buggy. For the closed system (buggy with one man + other man) from the conservation of linear momentum :
0 - (M + m) v* + m (u?+ v*)	(2)
Let v£ be the sought velocity of the buggy when the second man jump off the buggy; then from conservation of linear momentum of the system (buggy + one man) :
(M + m)v* «Mv^+m(u + v^ )	(3)
Solving equations (2) and (3) we get т(2М+Зт)ц*	...
2 ° (M+m)(M + 2m)	(ii) * * * * * 17
From (1) and (4) v2 - m « vx 2 (M + m)
Hence v2 > vt
1.157 The descending part of the chain is in free fall, it has speed v - V2 gA at the instant, all its points have descended a distance y. The length of the chain which lands on the floor during 1he differential time interval dt following this instant is vdt.
82
For the incoming chain element on the floor :	.
From dpy - Fv dt (where у - axis is directed down)	| 1 У
Q-(kvdi)v~ Fydt	£
or	Fy» -Xv2« -2Xgy	£5
Hence, the force exerted on the falling chain equals X v2 and is directed upward. Therefore	O\
from third law the force exerted by the falling	। S
chain on the table at the same instant of	— C? —>
2	К l
time becomes X v and is directed downward.	v оОСЭ* Y _
Since a length of chain of weight (Xyg) already lies on the table the total force on the floor is (2kyg) + (Xyg)« (3Xyg) or the weight of a length 3y of chain.
1.158 Velocity of the ball, with which it hits the slab, v « 'Jlgh v	1
After first impact, v' « ev (upward) but according to the problem v' = -, so e = - (1) and momentum, imparted to the slab, - mv - (- mv') = mv (1 + e)
Similarly, velocity of the ball after second impact, v" = ev' = e2 v
And momentum imparted « m (v\+ v" ) - m (1 + e) ev
Again, momentum imparted during third impact, - m (1 + e) e2 v, and so on, Hence, net momentum, imparted = mv (1 + e) + mve (1 + e) + mve2 (1 + e) + ...
 mv(l + e)(l + e + e2 + ...)
- mv > (from summation of G.P.)
m ^Jlgh / (r) + 1) / (т) - 1) (Using Eq. 1)
= 0-2 kg m/s. (On substitution)
1.159 (a) Since the resistance of water is negligibly small, 1he resultant of all external forces acting on the system “a man and a raft” is equal to zero. This means that the position of the C.M. of the given system does not change in the process of motion.
i.e. - constant or, Ar£ - 0 i.e.	0
or,	m	+ Ar^j + MA^ = 0
Thus,	т(Г" + 1)+мГ- 0, or,
m+M
(b) As net external force on “man-raft” system is equal to zero, therefore the momentum of this system does not change,
So,	0 - m[v* (t) + v$(t) ] +Mv^(t)
83
1.159 (a) Since the resistance of water is negligibly small, the resultant of all external forces acting on the system “a man and a raft” is equal to aero. This means that the position of the C.M. of the given system does not change in the process of motion.
i.e. r£ - constant or, ArJ« 0 i.e.	0
or,	+	Ar^ - 0
Thus,	m(T* + I) + MI« 0, or, I» -----------
m + M
(b) As net external force on “man-raft” system is equal to zero, therefore the momentum of this system does not change,
So,	0 = m [ v* (f) + v% (t) ] + M (f)
—> z 4	m vfr)	4
As v* (r) or v2 (r) is along horizontal direction, thus the sought force on the raft
_ Md^(t) _ Mm dv"(t) dt m + M dt
Note : we may get the result of part (a), if we integrate Eq. (1) over the time of motion of man or raft.
1.160	In the refrence frame fixed to the pulley axis the location of C.M. of the given system is described by the radius vector
Ar*-------------2M—1---------
But A^- -A^_M)
and	Ar> A	-«) + д Км-т)
Th	A - m
Thus ^C~2M
Note : one may also solve this problem using momentum conservation.
1.161	Velocity of cannon as well as that of shell equals V2 gl sin a down the inclined plane taken as the positive x - axis. From the linear impulse momentum theorem in projection form along x - axis for the system (connon + shell) i.e. Apx « Fx ist:
p cos a -M'J 2gl sin a - Mg sin a A t (as mass of the shell is neligible)
or	д t p cos a - A/V 2 gl sin a
’	Mg sin a
1.162	From conservation of momentum, for the system (bullet + body) along the initial direction of bullet
mv0 « (m + M) v, or,
m+M
84
1.163	When the disc breaks off the body M, its velocity towards right (along x-axis) equals the velocity of the body M, and let the disc’s velocity'in upward direction (along у-axis) at that moment be v’
From conservation of momentum, along x-axis for the system (disc + body)
mv = (m+M)v' or v' «	~	(1)
x x m+M	v '
And from energy conservation, for the same system in the field of gravity : j-mv2 = | (m + M) v'2 +	v’2 + mgh',
where h' is the height of break off point from initial level. So,
1	2	1 /	m2 v2 1	,2	.
-mv = 2{m+M)^mj+2mVy+m8h ’	USUlg (1)
,2 /2	2	л i /
or,	v y v ~~i------
y (m+M)
Also, if h" is the height of the disc, from the break-off point, then,	v'2 = 2 gh"
So,
w ,,2
Hence, the total height, raised from the initial level
,,	Mv2
= h+ h = -——----r
2g (M+m)
1.164 (a) When the disc slides and comes to a plank, it has a velocity equal to v = V2 gh- Due to friction between the disc and the plank the disc slows down and after some time the disc moves in one piece with the plank with velocity v' (say).
From the momentum conservation for the system (disc + plank) along horizontal towards right :
/	, mv
mv= (m+M)v or v = ------77
v	miM
Now from the equation of the increment of total mechanical eneigy of a system : I (M + m) v’2 -^mv2- Afr
or,	^(M+m)	m2v2 1 (m+M)2 2	mv2 = А^г
	1 2	Г	1 m	_ Л I
so,	— V	_,	~ m	ж
	2	M + m	fr
Hence,
A<’-
f 1	mM .	.
where a « -77 = reduced mass
m+M
85
(b) We look at the problem from a frame in which the hill is moving (together with the disc on it) to the right with speed u. Then in this frame the speed of the disc when it just gets onto the plank is, by the law of addition of velocities, v = и + V2gh. Similarly the common speed of the plank and the disc when they move together is
“**/O	1	9	1	9
Then as above - — (m+M)v - — mv - —Mu
- | (m + M)
1 , 1 .__________
- rfm + M) и -~m2u i2gh - mgh £ £
We see that Ад is independent of и and is in fact just - p g h as in (a). Thus the result obtained does not depend on the choice of reference frame.
Do note however that it will be in correct to apply *‘conservation of enegy” formula in the frame in which the hill is moving. The energy carried by the hill is not negligible in this frame. See also the next problem.
1.165	In a frame moving relative to the earth, one has to include the kinetic energy of the earth as well as earth’s acceleration to be able to apply conservation of energy to the problem. In a reference frame falling to the earth with velocity v0, the stone is initially going up with velocity v0 and so is the earth. The final velocity of the stone is 0 = vo - gt and
m
that of the earth is vo + — gt (M is the mass of the earth), from Newton’s third law, where t =
~ M- '
time of fall. From conservation of energy .	2
1	2	1 «г 2	»	1	™ l
-mv0 + -Mv0 + mgh = —M v0 + ^v0|
1 2 (	,
-v0 m + - - mgh
Hence
Negecting 77 in comparison with 1, we get M
Vo - 2gh or v0 -
The point is this in earth’s rest frame the effect of earth’s acderation is of order — And M
can be neglected but in a frame moving with respect to the earth the effect of earth’s acceleration must be kept because it is of order one (i.e. large).
1.166	From conservation of momentum, for the closed system “both colliding particles” + m2v^ = (m1 + m2) v*
-	1(3^2Л+2(4^бГ)	o л r*
mr + m2	3
Hence	|	= V 1 + 4 + 16 m/s = 4*6 m/s
86
1.167
For perfectly inelastic collision, in the C.M. frame, final kinetic energy of the colliding system (both spheres) becomes zero. Hence initial kinetic energy of the system in C.M. frame completely turns into the internal energy (Q) of the formed body. Hence
Q- |vT-i?|2
1	fr fr Э
Now from energy conservation A T- - Q « - ~ u I- v2| ,
In lab frame the same result is obtained as
АГ 1
A 1 - ™ -----------
2 m1 + m2
I i 2 I —2 2W1IV1I + m2 I v2 I
1.168
1	_►	_^2
(a) Let the initial and final velocities of m1 and m2 are ur, u2 and v, v2 respectively. Then from conservation of momentum along horizontal and vertical directions, we get:
= m2 v2 cos 0	(1)
and	m2v2sin6	(2)
Squaring (1) and (2) and then adding them, »»2V2=	+
Hr
Now, from kinetic energy conservation, 1	2 1	2 1	2
yW 2W2v2 + 2Wivi
or,
or,
(3)
m2
m (u^ - vj) - w2v2 - »»2 ~| («? + vj) [Using (3)] m2
V2
и2 1-
2 | m2 “ ml I ~ ml^m2
So, fraction of kinetic energy lost by the particle 1, 1	2 1	2
2т1и1-2т1*1 ЗВ ----
1
2 m2-ml 2mr mr + m2 m1^m2 (b) When the collision occurs head on, W1M1“ mlvl + m2v2 and from conservation of kinetic energy,
or,
(4)

1.21 A 2
M1
[Using (4)]
(1)
(5)
87
1	2	1	2	1	2
-/И1И1= 2mlvl + 2m2V2
W»1(«1-V1)2 "»2
or,
1 л 1
“ 2mivi + 2m2
[Using (5)]
v fai/mi-l) or	— =
’	ur
Fraction of kinetic eneigy, lost
\2	A
mi ~ I	4 mi m2
+	’ (m1 + m2)2
[Using (6)]
(6)
1.169 (a) When the particles fly apart in opposite direction with equal velocities (say v), then from conservatin of momentum,
m1 и + 0 « (m2 - mJ v	(1)
and from conservation of kinetic eneigy,
1	2	1	2	1	2
- 2W1V +2m2V or,	mru2^ (m^ + mjv2	(2)
From £q. (1) and (2), 2 2	yA-*'
2	miu	л
mru •	+	„ Z-J
(m2-mx)	—\&=60°
or, m2 - 3 mx m2« 0	r~*\
mi 1 Hence —« — as и 0 m2 3	2
(b) When they fly apart symmetrically relative to the initial motion direction with the angle of divergence 0 » 60°, From conservation of momentum, along horizontal and vertical direction,
cos (0/2) + m2 v2 cos (0/2)	(1)
and	mx Vi sin (0/2) ® v2 sin (0/2)
or,	mr vx - m2 v2
Now, from conservation of kinetic eneigy, 1	2 л 1	2	1	2
-WiUi + 0- yWi vj-t-y
From (1) and (2), /	mi vi \
mr ut« cos (0/2) 0*1 vx +----m2!ж 2 vr cos (0/2)
(2)
(3)
88
So,	- 2 Vj cos (0/2)
From (2), (3), and (4)
4mico.2(e/2)v;. «2
,	m,
or, 4 cos2 (0/2)  1+ —
/^2
ml .	2 0 i
or,	—« 4cos — -1
«2	2
(4)
ml
and putting the value of 0, we get, —  2 «2
1.170 If (vb,vly) are the instantaneous velocity components of the incident ball and ( Vqx > v2y) are velocity components of the struck ball at the same moment, then since there are no external impulsive forces (i.e. other than the mutual interaction of the balls) We have	и since 	, v2y “ 0
m и cosa = m + m
The impulsive force of mutual interaction satisfies d ( . F d f . dr Vbc ~ m ~ dt^1^
( F is along the x axis as the balls are smooth. Thus Y component of momentum is not transferred.) Since loss of К.Б. is stored as deformation energy D, we have
1	2 1	2 1	2
2	2	1	2 2
I 2	2	1	2 1	2
- — mu cos a -	-^mv^r
2	2 u 2
I Г 2 2	2	22/	\ 2 1
“ 5— m u cos a “ m vix ~ (mweosa- mv^)
= — £ Zm^cosav^ - 2m2v^ j = m (v^ucosa - v^2)
2
2	2/	\
u cos a / и cosa \
-m[—T—	vbj
We see that D is maximum when
и cosa
89
1171 From the conservation of linear momentum of the shell just before and after its fragmentation ЗГ-iT+^+i^	(1)
where , v2 an<^ v3 are the velocities of its fragments.
From the energy conservation 3r)v2 - v2 + v2 + v3	(2)
£S^	•ф W
Now	v- or * v£ - vc - vf. - v	(3)
where = v*= velocity of the C.M. of the fragments the velocity of the shell. Obviously in the C.M. frame the linear momentum of a system is equal to zero, so
i£+i£+i£-0	(4)
Using (3) and (4) in (2), we get
3r]v2 - (v + vj )2 + (v + v£)2 + (Г-	i>2 )2 - 3V2 + 2v 2 + 2v 2 + 2 ’ ^2
or,	2v i + 2vt v2cos0 + 2v2 + 3 (1 -r])v2 = 0	(5)
If we have had used « - v^~ V3, then Eq. 5 were contain v3 instead of v2 and so on. The problem being symmetrical we can look for the maximum of any one. Obviously it will be the same for each.
For v^o be real in Eq. (5)
4 v 2 cos20 at 8(2v 2 + 3 (1 - tj) v2) or 6(r) - l)v^ at (4 - cos20)v 2
SO,	V2< V	0Г	V
▼ 4-cos0
Hence v2(max)- |v +	-v + V2(r]-l) v - v(l + V2(vj- 1) j- 1 km/s
Thus owing to the symmetry
Vl(max) “ V2(max) = V3(max) ’ V (* + ^2(1] - 1)) = 1 km/s
1172 Since, the collision is head on, the particle 1 will continue moving along the same line as before the collision, but there will be a change in the magnitude of it’s velocity vector.
Let it starts moving with velocity and particle 2 with v2 after collision, then from the
conservation of momentum
mu « mvr + mv2 or, и =	+ v2
And from the condition, given,
,2 J
T)-	- 1	-------
or, From (1) and (2), or,
(1)
vl + v%- (I-tj)m2
Vi + (и - vj2 - (1 - n) “2 v? + u2 - 2 uv, + v? - (1 - тй u2
(2)
90
or,	2v2-2v1m + t]m2» 0
o	„ V4m2-8t)m2
So,	Vi - 2м ±-----—1—
= 2 [ M ± Vm2-2t] M2 j = ~ и (1 ± VI - 2r] )
Positive sign gives the velocity of the 2nd particle which lies ahead. The negative sign is correct for .
So, v1 = “M (1 - VI - 2t) ) = 5 m/s will continue moving in the same direction.
Note that vx = 0 if т)« 0 as it must.
1.173 Since, no external impulsive force is effective on the system “M + m”, its total momentum along any direction will remain conserved.
So from px - const.
mu « Mv. cos 0 or, » -77 —“7Г	(1)
1	1 M cos 0	v 7
and from py - const
M
mv2 = MvY sin 0 or, v2 = — sin 0 = и tan 0, [using (1)]
Final kinetic energy of the system
1	2
And initial kinetic energy of the system» mu
So, % change =	— x 100
and putting the values of 0 and — , we get % of change in kinetic energy» - 40 %
1.174 (a) Let the particles mr and m2 move with velocities v1 and respectively. On the basis of solution of problem 1.147 (b)
91
As	Vi X v2
~ Го-------T	m7
So,	p» pVvT + vi where p- --------
mr + m2
(b) Again from 1.147 (b)
2И^“ 2И Г1’^ |
So,	T-|h(v* + $
1.175 From conservation of momentum —*	—
Pi e Pi + Pi
so	(л--Pi ) “ Pi" 2Л Pl COS0! + Pl2 • p2' 2
From conservation of eneigy
„2	„	,1	/2
Pl	Pl	Pl
2mx	2	2m2
Eliminating p2 we get
J	m2 1	21	m2
° - Pi 1 + — - IPiPiCosdi +Л 1 - —
I	fflj 1	I
This quadratic equation for pr' has a real solution in terms of pr and cos 0г only if
mi sin 0. m -----
ml
1.176 From the symmetry of the problem, the velocity of the disc A will be directed either in the initial direction or opposite to it just after the impact Let the velocity of the disc A after the collision be V and be directed towards right after the collision. It is also clear from the symmetry of problem that the discs В and C have equal speed (say v") in the directions, shown. From the condition of the problem, d
T| у	•______
cos 0  -j- - so, sin 0 - V4-n2 / 2	(1)
For the three discs, system, from the conservation of linear momentum in the symmetry direction (towards right)
mv« 2m v"sin0 + mv' or, v « 2 v" sin 0 + v'	(2)
92
From the definition of the coefficeint of restitution, we have for the discs A and В (or C)
v" - v' sin 0 e _. —-----------
v sin 0 - 0
But e = 1, for perfectly elastic collision,
So, v sin 0 = v" - v' sin 0
From (2) and (3), , _ v (1 ~ 2 sin2 0)
V ~ (1 + 2 sin2 6)
=	~	{using (1)}
6 - T|
Hence we have,
Therefore, the disc A wdl recoil if r| < V2 and stop if rj = V2.
Note : One can write the equations of momentum conservation along the direction perpendicular to the initial direction of disc A and the consevation of kinetic energy instead of the equation of restitution.
1.177 (a) Let a molecule comes with velocity to strike another stationary molecule and just after collision their velocities become and v*2 respectively. As the mass of the each molecule is same, conservation of linear momentum and conservation of kinetic energy for the system (both molecules) respectively gives :
Л + ^2
and
2	/2	,2-
Vl = Vl+V2
From the property of vector addition it is obvious from the obtained Eqs. that
V ! JL V 2 or V } ’ V 2 = 0
(b) Due to the loss of kinetic energy in inelastic collision v2 > v'2 + v'2
so, v {•	> 0 and therefore angle of divergence < 90°.
1.178 Suppose that at time tf the rocket has the mass m and the velocity v*, relative to the reference frame, employed. Now consider the inertial frame moving with the velocity that the rocket has at the given moment. In this reference frame, the momentum increament that the rocket & ejected gas system acquires during time dt is,
dp*= md v + ц dtu*= F dt
t/v*	-+
or,	m ~r~ = F - ц и
dt
or,	m w = F - ц и
93
1.179
According to the question, F «
Oandp,  - dm/dt so the equation for this system becomes,
dv dm т-_я —u dt dt
As d iZf | iZ so, m dv  - и dm. Integrating within the limits :
v , or “ = In — и m
m0 Thus, v= «In — m
-к	->	—> mo
As d vf | u. so in vector form v « - и In — m
1.180 According to the question, F (external force) = 0
As	dv*	н «Г
so, in scalar form,	m dv »	-и dm
wdt	dm or,				 и	m Integrating within the limits for m (r) ж	
wt _ _ Г dm и J m	v , m or, — = -In — м	m0
Hence,	m= mQe
1.181
As F « 0, from the equation of dynamics of a body with variable mass;
dv* -*dm	-+dm
m -7- * и —г or, d v « и — dt dt	m
Now t/iZf jirand ?ince iZl v^we must have | dv*\ = voda (because v0 is constant)
Where J a is the angle by which the spaceship,turns in time dt.
dm , j и dm
-u —» vnda or, da» ----------------
m u	v0 m
So,
(1)
m
u I dm и , (mo or,	a »----I — » — In I —
v0 J m V0 \m

94
1.182
We have = - pt or, dm = - pi dt dt
dm « - pi I dt or, m « mQ - pu о
As u*= 0 so, from the equation of variable mass system :
/ ч dv =* dv -» z* 4 (m0-\U) — =F or, — = F/(m0-fit)
Integrating
V
1.183
or,
Г,-* 7* I dt
I dv - F I 7-------г
f	J	(ff»o - (Я)
о 0
F.	1
— In -------
H - H* J
Let the car be moving in a reference frame to which the hopper is fixed and at any instant of time, let its mass be m and velocity v. Then from the general equation, for variable mass system. d v*	-*dm
m T“e F + u — dt	dt
Hence
We write the equation, for our system as,
Jv* z* -*dm	-
m — = F - v — as, и = - v dt	dt
(1)
So
(mv) = F dt
and
-> Ft .	.
v » — on integration.
m
But
m » m0 + pt
so,
Ft
mQ 1 + J— mo
-> dv F
Thus the sought acceleration, w « —
dt /1 И m0 1 + — mo
1.184 Let the length of the chain inside the smooth horizontal tube at an arbitrary instant is x. From the equation, -> z* -^dm
mw » F + u —7-dt
95
as и ~ 0, F f f w, for the chain inside the tube
1	'Г U 1 m
Kxw^T where A= —
Similarly for the overhanging part, 0
Thus
mw = F
(1)
W
or khw = "khg-T From (1) and (2),
(2)
к (x + ti) vv = khg or, (x + Л) v ~-as
hg
or,
dv .
- gh,
(-Л)
[As the length of the chain inside the tube decreases with time, ds = - dr.]
vdv= -2П------Г-
х + Л
or,
Integrating,
о
f dx
0
(2-Л)
or,
v2 (i\	!	77
у = gh In l-l or v = у 2g/i In -
1.185 Force moment relative to point О ;
N = 2bt dt
—->	—>
Let the angle between M and N, a = 45° at
71,611 72
t ~ to >
M-tl («**• fo): (2 fap) |Л/| |ЛГ| “ Va2 + 62t04 2bt0
2*^o
о
btf
2 + b2t*
So, 2 b2104 » a2 + b2 or, r0 «
It is also obvious from the figure that the angle a is equal to 45° at the moment r0, when a-b r02, i.e. r0 = ^a7b and N = 2 b.
96
1.186
-> -> -> /-> “ 1 > A/(r) =	vot + -gf Ixm
2
= mvQ gt sm
1	2 • fa \
+ 2 sm —+ а (к )
1.187
1.188
1	2	->
-mvQgt cosa(-£ ):
mvagt2 cos a Thus M(t) °
i.e. at
2
Thus angular momentum at maximum height т v0 sin a xs — = -------------
2 g ’
I V 1 Im Vo1 2	2
M I— » |-t— sin a cos a « 37 kg - m/s \2/ \ 2S /
Alternate :
M (0)« 0 so,
о
(_fr 1 _fr 2 \	_J
vof + -gr Ixmg dt
0
2
о \	f J
(a)	The disc experiences gravity, the force of reaction of the horizontal surface, and the force R of reaction of the wall at the moment of the impact against it. The first two forces counter-balance each other, leaving only the force R. It’s moment relative to any point of the line along which the vector R acts or along normal to the wall is equal to zero and therefore the angular momentum of the disc relative to any of these points does not change in the given process.
(b)	During the course of collision with wall the position of disc is same and is equal to Obviously the increment in linear momentum of the ball Ap*« 2mv cos a n Here, АЛ/ = r\ x Ap*= 2 mv I cos a n and directed normally emerging from the plane of figure
Thus | A M | « 2 mv I cos a
(a) The ball is under the influence of forces T and m g*at all the moments of time, while moving along a horizontal circle. Obviously the vertical component of Tbalance mg and
97
so the net moment of these two about any point becoems zero. The horizontal component —♦
of T, which provides the centripetal acceleration to ball is already directed toward the centre (C) of the horizontal circle, thus its moment about the point C equals zero at all the moments of time. Hence the net moment of the force acting on the ball about point C equals zero and that’s why the angular mommetum of the ball is conserved about the horizontal circle.
(b) Let a be the angle which the thread forms with the vertical.
Now from equation of particle dynamics :
T cos a » mg and T sin a » mco21 sin a
Hence on solving cos a = -4-	(1)
co I
—->
As | M | is constant in magnitude so from figure. — »
| AM|« 2Mcos a where
M- |Ц.|- |MZ| •
-	mvl^s
Thus) AM | = 2mvZcosa= 2 mail2 sin a cos a
1.189 During the free fall time t - т
the reference point О moves in hoizontal direction
(say towards right) by the distance Vt. In the translating frame as M (O)- 0, so
AM= Mf~ 7* —*• —*	—> —*
- (-Vti + Aj )*m[gxj - Vi ]
« -m V gx? h +mVh(+k)
-----
Yj (9)
Hence
ж -m Vg(—^Г+даУЛ(+Г) - -mVhk l S j
| AM | « mVh
1.190 Coriolis force is. (2m v* x of).
Here ccT is along the 2-axis (vertical). The moving disc is moving with velocity v0 which is constant. The motion is along the x-axis say. Then the Coriolis force is along y-axis and has the magnitude 2m v0 co. At time t, the distance of the centre of moving disc from О is VqT (along x-axis). Thus the torque N due to the coriolis force is
N » 2m v0 co-VqT along the z-axis.
98
Hence equating this to
dM -2	> ж 2	2
2mv0cot or M = mvQwt + constant.
The constant is irrelevant and may be put equal to zero if the disc is originally set in motion from the point O.
This discussion is approximate. The Coriolis force will cause the disc to swerve from straight line motion and thus cause deviation from the above formula which will be substantial for large r.
1.191 If r « radial velocity of the particle then the total .energy of the particle at any instant is b?2+S+fo-2=£	(i)
where the second term is the kinetic energy of angular motion about the centre O. Then
the extreme values of r are determined by r = 0 and solving the resulting quadratic equation Л(г2)2-£г2 + ^ = 0
we get
„ -/„2 2Mzk лЕ±УЕ -~пГ-
2k
From this we see that
£-Л(г2 + г2)	(2)
where is the minimum distance from О and r2 is the maximum distance. Then
+ ^^2 "	+ r2 )
2kr2
Hence,	m « —y-
V2
•Note : Eq. (1) can be derived from the standard expression for kinetic energy and angular momentum in plane poler coordinates :
Т» ~mr2 + r2 0 2
2	2
M = angular momentum = mr2 0
1.192 The swinging sphere experiences two forces : The gravitational force and the tension of the thread. Now, it is clear from the condition, given in the problem, that the moment of these forces about the vertical axis, passing through the point of suspension Nz - 0. Consequently, the angular momentum Mz of the sphere relative to the given axis (z) is constant Thus	mvo(/sin0)« mvl	(1)
where m is the mass of the sphere and v is it 5 velocity in the position, when the thread forms an angle у the vertical. Mechanical energy is also conserved, as the sphere is
99
under the influence if only one other force, i.e. tension, which does not perform any work, as it is always perpendicular to the velocity.
1 о	1 о
So,	у mvQ + mg Z cos 0 = у mv	(2)
From (1) and (2), we get,
v0= V2g//cos 0
1.193	Forces, acting on the mass m are shown in the figure. As N = mg, the net torque of these two forces about any fixed point must be equal to zero. Tension T, acting on the mass m is a central force, which is always directed towards the centre O. Hence the moment of force T is also zero about the point О and therefore the angular momentum of the particle m is conserved about O.
Let, the angular velocity of the particle be co, when the separation between hole and particle m is r, then from the conservation of momentum about the point O, :
«(®oro)ro* m(a>r)r,
Now, from the second law of motion for m, T= F = m($2r
Hence the sought tension;
m Wq Tq r	m coq Tq
1.194	On the given system the weight of the body m is the only force whose moment is effective about the axis of pulley. Let us take the sense of co of the pulley at an arbitrary instant as the positive sense of axis of rotation (z-axis)
As	K(0)-0, so,	f^dt
Z y	'	z	£	' ' J a*
So,
(0 » I mgR dt= mgRt
о
1.195	Let the point of contact of sphere at initial moment (t= 0) be at O. At an arbitrary moment, the forces acting on the sphere are shown in the figure. We have normal reaction N = mg sin a and both pass through same line and the force of static friction passes through the point O, thus the moment about point О becomes zero. Hence mg sin a is the only force which has effective^torque about point O, and is given by mgR&ina normally emerging from the plane of figure.
As M (t = 0) = 0, so, AM = M(f) = f N dt
Hence, M (t) - Nt • mgR sin at
100
1.196	Let position vectors of the particles of the system be r*and r* with respect to the points О and O' respectively. Then we have,
0)
where rj is the radius vector of O' with respect to O.
Now, the angular momentum of the system relative to the point О can be written as follows;
M “ 2	+ S	[using CD]
or,	M= M + (fyxp^, where, jT= pf	G)
From (2), if the total linear momentum of the system, p*= 0, then its angular momentum does not depend on the choice of the point O.
Note that in the C.M. frame, the system of particles, as a whole is at rest.
1.197	On the basis of solution of problem 1.196, we have concluded that; “in the C.M. frame, the angular momentum of system of particles is independent of the choice of the point, relative to which it is determined” and in accordance with the problem, this is denoted by M.
We denote the angular momentum of the system of particles, relative to the point O, by M. Since the internal and proper angular momentum in the C.M. frame, does not depend on the choice of the point O', this point may be taken coincident with the point О of the X-frame, at a given moment of time. Then at that moment, the radius vectors of all the particles, in both reference frames, are equal ( rf = *7) an^ the velocities are related by the equation,
V*= V . +	,	(1)
where is the velocity of C.M. frame, relative to the X-frame. Consequently, we may write,
X^) + S
or, M = $+m	as	where m =
or,	M-	=
1.198	From conservation of linear momentum along the direction of incident ball for the system consists with colliding ball and phhere
mvo-mv+yvj	(1)
where v' and are the velocities of ball and sphere 1 respectively after collision. (Remember that the collision is head on).
As the collision is perfectly elastic, from the definition of co-efficeint of restitution, v' - vx
1 “ ‘0^’ or’ v' ' V1 ’ ' v0	(2)
101
Solving (1) and (2), we get,
4 v0
V1" ~~зГ’ directed towards right.
In the C.M. frame of spheres 1 and 2 (Fig.)
Л * -P2 and 1л I “ 1^ I ’ Hlh-Vjl
Also, r^c = -r^., thus Й = 2[t\c*p[]
. — , =* л ,[/ m/2 4v0 л' Asric-LA> so, .10-2 - —---3~"
(where n is the unit vector in the sense of r\c )
~ mvd
Hence M=—£~
1.199 In the C.M. frame of the system (both the discs + spring), the linear momentum of the discs are related by the relation, Pi * at all the moments of time, where, And the total kinetic energy of the system,
T = i ц	[See solution of 1.147 (b)]
Bearing in mind that at the moment of maximum deformation of the spring, the projection of along the length of the spring becomes zero, i.e. - 0.
The conservation of mechanical energy of the considered system in the C.M. frame gives. 1 (m\ 2 1	2 1 (m\ 2
2(2^°* 2KX +2^2^1^Ь’>
Now from the conservation of angular momentum of the system about the C.M., 1	(lo + x} m
2^2Д2 V°J’ 2( 2 ) 2 Vrel<y)
volo L x\ 1 Л x \	,	...
“	'-«(И'Ч'Ч -V» l-j- ..SX«/O	(2)
Using (2) in (1),
1	2
2mV^
KX2
or,
|mvg 1-11-
2
or,
As
'o /02
- к x2, [neglectingx2/
4)
m Vq хи 0, thus x = —=-k/0
102
1.4
UNIVERSAL GRAVITATION
1.200
We have
ATv2 yMm, ----ж —— or
Y^
V2
Thus
v
V CD » — « ---
r imjv2
v3
(Here ms is the mass of the Sun.)
2лx6-67x 10-11 x 1-97x1030	,	,п1	_
----г-2 - 	Г5--------------------- 1-94 х 10 sec - 225 days. v3	(34-9 х 103)3
(The answer is incorrectly written in terms of the planetary mass M)
So
1.201 For any planet
MRm2-
yMms --— or CD
R2
So,

2 71
CD
(a) Thus
Tj
'tf2
A
So
(Tj/Te)2/3~ (12)2/3- 5-24.
ЛЕ
2/3
(b)
and A
So
V2	v рлутЛ2"3
VJ----------- or> VJ -1 ~T~~
where T« 12 years. ms - mass of ths Sun.
Putting the values we get Vj « 12*97 km/s
2	/~	ч273	2/3
vj	(2 л у m5 \	/ 2 л	\
Acceleration = — » -------— x I —7= |
RJ	\ J )	\TVym I
4/3
,1/3
2-15 x 10-4 km/s2
103
1.202 Semi-major axis» (r + R)/2
It is sufficient to consider the motion be along a circle of semi-major axis
r+R
2
for T
does not depend on eccentricity.
у - v 3/2
2 л I 2 I
Hence	T = ---V --------« л V(r + R)3/2yms
(again ms is the mass of the Sun)
1.203	We can think of the body as moving in a very elongated orbit of maximum distance R and minimum distance 0 so semi major axis = R/2. Hence if т is the time of fall then
2t? (R/2\3
— ” -7Г"	ОГ
г я
т2- T2/32
or	t = Т/4^2 = 365 / 4V2 - 64-5 days.
1.204	T= 2nR3/2/Vym^
If the distances are scaled down, Я3/2 decreases by a factor T)3/2and so does ms . Hence T does not change.
m2
1.205	The double star can be replaced by a single star of mass-moving about the centre
/И1 + m2
of mass subjected to the force у mlm2/ r2. Then
So
or,
т	2лг3/2	2лг3/2
j /	^2
V у m.m2 —---------
v 1 2 mx + m2
r3/2-
2 л
2/3	----------
Г • T- (YW)173 - v у M (T/2 л)2 I Z Л I
1.206 (a) The gravitational potential due to m1 at the point of location of m2 ;
ymr m2

So,
^21 ж m2 ^2 "
Similarly
1Z	«
104
Непсе
пи и21~ и- У—р
7ПГ	П1г
6/Х
(Ъ) Choose the location of the point mass as the origin. Then the potential erfergy dU of M
an element of mass dM • -jdx of the rod in the field of the point mass is
dU^-vm -rdx —
1	I x
where x is the distance between the element and the point (Note that the rod and the point mass are on a straight line.) If then a is the distance of the nearer end of the rod from the point mass.
И
dx
>7П
гт тМ I dx M. । U « — v—— I — « - vm— In
I J a x / I
The force of interaction is
da
тМ I
J_\ ymM a2 I a(a + l)
a
Minus sign means attraction.
1.207 As the planet is under central force (gravitational interaction), its angular momentum is conserved about the Sun (which is situated at one of the focii of the ellipse)
2
So,	mv2r2 or, vt« —j-	(1)
From the conservation of mechanical energy of the system (Sun + planet), i 2 ymsm i 2 -------------------------+ —mv~--------------+ mv
Г1 2	1 r2 2	2
or,	— + 7^4° ~Г7“ +7v2 [Using(l)]
rl L	\'2 )	£
Thus,	v2- y!2^msrjr2(rv + r^	(2)
Hence	mv2r2- m V2 у r2 / (fj +
105
1208 From the previous problem, if rt , r2 are the maximum and minimum distances from the sun to the planet and , v2 are the corresponding velocities, then, say, F_1 v2 S
E - r2 ynvn гл ymrn	vmmr	ymrn
--------------------------hr1 [Using Eq. (2) of 1.207]
Г1 + r2 r2 r2	rr + r2 2a L &	/ j
where 2d = major axis « rt + r2. The same result can also be obtained directly by writing an equation analogous to Eq (1) of problem 1.191.
_ 1 .2 M2 Ymms E = ~mri-------z-----
2 2mr2 r
(Here M is angular momentum of the planet and m is its mass). For extreme position
r « 0 and we get the quadratic
The sum of the two roots of this equation are ri + r2’s-'LF£’s2a
1 Z	£
£-—-—« constant
Thus
1209 From the conservtion of angular momentum about the Sun. mvorosince»	mv2r2 or, v2r2« v0r2sina
From conservation of mechanical energy, 1 2
'о 2	1	'•i
Vn>nSin2a ym, 2rj q
(1)
or,
1 2 Чт*т
vo Y^
2 r0
or,
2 2
ms ri ~ vo ro s*n a “ 0
So,
'22.2
У0фт a) *o- Го
i _ 0 0
Y^
r0 J 1 ± V 1 - (2 - T|) T) sin2 a
(2-n)
where T| =	f0 / у ms, (ms is the mass of the Sun).

/9 2 у m"
2 r
2 _ A' (ro ~
• 2
106
1.210	At the minimum separation with the Sun, the cosmic body’s velocity is perpendicular to its position vector relative to the Sun. If r^n be the sought minimum distance, from conservation of angular momentum about the Sun (C).
v0/
mvol- mvrmin or, v- —	(1)
Gnin
From conservation of mechanical energy of the system (sun + cosmic body),
So,	1	2	i 2 2	’	'’min + 2 2		 2 vo	Y ms vo	
	2	r 2r2	(	8 } mm "min	
or,	v02r^n + 2Yff’Irmiii-v02/2- °	
So,	-2Ymf ±V4y2m2 + 4v02 v02 l2	/ y2m2 + Vgl2
	Гта	2v2	Vo2
Hence, taking positive root
'’min- (Y»»,/’,o2)[Vl + (/vo2/Ym,)2 -1]
1.211	Suppose that the sphere has a radius equal to a. We may imagine that the sphere is made up of concentric thin spherical shells (layers) with radii ranging from 0 to a, and each spherical layer is made up of elementry bands (rings). Let us first calculate potential due to an elementry band of a spherical layer at the point of location of the point mass m (say point P) (Fig.). As all the points of the band are located at the distance I from the point P, so,
d ф « - (where mass of the band)	(1)
dM = }(2nasin6)(adB)
I 4л a j
»	(2)
And Z2- a2 + r2 - 2arcos0 (3) Differentiating Eq. (3), we get Idl- ar sinOJO	(4)
Hence using above equations
I V dM\
<5>
107
Now integrating this Eq. over the whole spherical layer ria r-a
So	dip-	(6)
Equation (6) demonstrates that the potential produced by a thin uniform spherical layer outside the layer is such as if the whole mass of the layer were concentrated at it’s centre; Hence the potential due to the sphere at point P\
<p-J*d<p-	(7)
This expression is similar to that of Eq. (6)
Hence thte sought potential eneigy of gravitational interaction of the particle m and the sphere,
rr	yMm
U > mcp - ~ J-
(b) Using the Eq.,
dtp “ dr
O-
G..-2L
(using Eq. 7)
So	u’-	(8)
r	r
1.212 (The problem has already a dear hint in the answer sheet of the problem book). Here we adopt a different method.
Let m be the mass of the spherical layer, wich is imagined to be made up of rings. At a point inside the spherical layer at distance r from the centre, the gravitational potential due to a ring element of radius a equals,
dcp - - dl (see Eq. (5) of solution of 1.211)
So, (p « f dtp « - I dl« -’ v J Y 2ar J a
(1)
Hence
G •	0 .
r dr
Hence gravitational field strength as well as field force becomes zero, inside a thin sphereical layer.
1.213 One can imagine that the uniform hemisphere is made up of thin hemispherical layers of radii ranging from 0 to R. Let us consider such a layer (Fig.). Potential at point O, due to this layer is,
108
, ydm ЗуМ *	,	, M ( 4лг2>\ ,
а ф = -J~~г~ гаг у where dm = ------------т —-— аг
Г R3	(2/3)лЯЦ 2 )
(This is because all points of each hemispherical shell are equidistant from O.) к
Hence, ф« [dtp- -	f rdr« -	. .
J * R3 Jo 2R / V/XM
Hence, the work done by the gravitational field /	\S\A\
force on the particle of mass m, to remove it / jA \\ \ to infinity is given by the formula	q [ j | |	"	>
A = mcp, since ф « 0 at infinity.	1	\ I II ]
Hence the sought work,	\	\Z/ /
_ 3yjnM_ \
0 -00 2K \^J
(The work done by the external agent is - A.)
1.214 In the solution of problem 1.211, we have obtained ф and G due to a uniform shpere, at a distance r from it’s centre outside it We have from Eqs. (7) and (8) of 1.211,
Ф « - and G « - Г	(A)
Accordance with the Eq. (1) of the solution of 1.212, potential due to a spherical shell of radius a, at any point, inside it becomes
Ф« Const, and G «	О	(B)
v a	r dr	v 7
For a point (say P) which lies inside the uniform solid sphere, the potential ф at that point may be represented as a sum.
Ф. = ф1 + ф2 inside
where фх is the potential of a solid sphere having radius r and ф2 is the potential of the layer of radii r and R. In accordance with equation (A)
у ( M 4 з\ yM 2 ф = _м------------г-л г =
1	Ц(4/3)лЯ33 j R3
The potential ф2 produced by the layer (thick shell) is the same at all points inside it. The potential ф2 is easiest to calculate, for the point positioned at the layer’s centre. Using Eq. (B)
R
JdM 3 yM/n2 2x
where dM =
M .	2 ,	(3M\ 2 .
-------j 4 л r dr - —r dr (4/3)nR3	)
is the mass of a thin layer between the radii г and r + dr.
Thus
Ф. = <Pi + <P2 = inside
(O
109
From the Eq.
or
G -r dr
r R3
yM —	4
G~ -^r- -YT^pr
A	J
(where p =
M 4 _d3 3 R
, is the density of the sphere)
(D)
The plots cp (r) and G (r) for a uniform sphere of radius R are shown in figure of answersheet. Alternate : Like Gauss’s theorem of electrostatics, one can derive Gauss’s theorem for
gravitation in the form у G*dS* - 4 я у . For calculation of G at a point inside the sphere at a distance r from its centre, let us consider a Gaussian surface of radius r, Then,
A 2 A (3	~ yM
G„4jih« -4лу —r r or, G « -стг
r	r R3
Hence, G«	-y^jtpr*(as p« ---——t
R3 r3 H I K (4/3) kR3 J?	'	00
00
So, cp = f G dr = frdr+ f -^-^f-dr
J r Jr3 Jr2
T	r	R
Integrating and summing up, we get,
<p-
yM 2R
And from Gauss’s theorem for outside it :
Gr4nr2 = ~4луМ
n Iм or Gr« --4-
Thus	cp (r) - J Grdr~ -
r
1.215	Treating the cavity as negative mass of density - p in a uniform sphere density + p and using the superposition principle, the sought field strength is :
G ж Gj + G2
—>	4	—♦ д	—>
or G« - jJtypr+ + -	(- p) r.
(where r\ and r_ are the position vectors of an orbitrary point P inside the cavity with respect to centre of sphere and cavity respectively.) -♦4 r »	4
Thus G = - j л у p	- j л у p I
110
1.216	We partition the solid sphere into thin spherical layers and consider a layer of thickness dr lying at a distance r from the centre of the ball. Each spherical layer presses on the layers within it The considered layer is attracted to the part of the sphere lying within it (the outer part does not act on the layer). Hence for the considered layer
dp4xr2* dF
(4	3 \
У13 Г P 1(4лг2«/гр)
or, dP4nr2* ----------Ч5---------
r2
(where p is the mean density of sphere)
or, dp* jftyp2rdr
R
Thus p* J* dp* ~^yp2(P2-r2)
(The pressure.must vanish at r = R.)
3/
°r> Р-g
1 -(г2/Я2))уЛ/2/яЯ4, Putting p- M/(4/3) nR3
Putting r * 0, we have the pressure at sphere’s centre, and treating it as the Earth where mean density is equal to p * 5*5 x 103 kg/m3 and JR « 64 x 102 km we have, p * 1-73 x 1011 Pa or 1-72 x 106 atrns.
1.217
(a)	Since the potential at each point of a spherical surface (shell) is constant and is equal
to <p « - [as we have in Eq. (1) of solution of problem 1.212] К
We obtain in accordance with the equation U-	dm
2
1 { ym\ ym
2 Г R ~ 2R
(The factor ~ is needed otherwise contribution of different mass elements is counted twice.)
3 ym / r2 \
~ 2R ( 3P2J
elementry spherical layer confined between the radii
(b)	In this case the potential inside the sphere depends only on r (see Eq. (C) of the solution of problem 1.214)
Ф-
Here dm is the mass of an r and r + dr :
dm*
(4 я r 2 dr p) -
Ill
и « IJ dm Ф
я
After integrating, we get
u-5 R
1.218 Let co - у —3— « circular frequency of the satellite in the outer orbit,
co0-
чмЕ
(r - A r)3
circular frequency of the satellite in the inner orbit
So, relative angular velocity - co0 ± co where - sign is to be taken when the satellites are moving in the same sense and + sign if they are moving in opposite sense.
Hence, time between closest approaches
2л____________2 л__________1	/ 4-5 days (6 - 0)
= “0±	+ S ” I h°Ur 0 “ 2)
where 6 is 0 in the first case and 2 in the second case.
1.219
ю1-
yM
IF
6-67 x 10"11 x 5-96 x 1024
(6-37 x 106)2
= 9-8 m/s2
2	2
-J 6-37X 10е - 0-034 m/s2
2	T I 24 x 3600 x 7
and
co3-
yMs
R lxmean
6-67 x 10~u x 1-97 xlO30 (149-50 x 106 x 103)2
- 5- 9x 10-3 m/s2
Then
coj: co2 : co3 - 1: 0-0034: 0-0006
1.220
Let h be the sought height in the first case, so 99 yM 100 gw (Я + Л)2
yM	g
*	2 "	2
H’l
112
or
99	/	h\2
100“ Л
From the statement of the problem, it is obvious that in this case h«R
99	/	2h\	, R /6400V
l--^* or ft- —« "rr" km» 32 km
100 I R I 200 I 200 I
In the other case if h' be the sought height, than
g л k\2 1 Л hL\2
2" g 1 + Л or 2" 1 + л
Thus
From lhe language of the problem, in this case h’ is not very small in comparison with R. Therefore in this case we cannot use the approximation adopted in the previous case.
/	h’\2	К
Here, l+~ =2 So, ^--±V2-1 (	RI	R
As - ve sign is not acceptable
ft'- (vT-l)jR- (V2-1)6400km - 2650km
1.221 Let the mass of the body be m and let it go upto a height h. From conservation of mechanical energy of the system
Y Mm 1	2
- R +2/nv0«
1.222
• Iм Using =
g, in above equation and on solving we get,
R% 2gR-vl
Gravitational pull provides the required centripetal acceleration to the satelite. Thus if h be the sought distance, we have 2 m v ymM 7г—~	------т or,
(К + Л) (R + ft)2
SO,
(fl + ft)v2- yM
or.
Rv2 + hv2 = gR2,
yM 3S
-yMm л тЬтг0
v2	V2
1.223 A satellite that hovers above the earth’s equator and corotates with it moving from the west to east with the diurnal angular velocity of the earth appears stationary to an observer on the earth. It is called geostationary. For this calculation we may neglect the annual motion of the earth as well as all other influences. Then, by Newton’s law,
yMm I 2л \
r2 - W T Г
113
where M = mass of the earth, T = 86400 seconds = period of daily rotation of the earth and r = distance of the satellite from the centre of the earth. Then
Substitution of M = 5-96 x 1024 kg gives
r- 4-220 x IO4 km
The instantaneous velocity with respect to an inertial frame fixed to the centre of the earth at that moment will be
( )r“ 307 km/s
and the acceleration will be die centripetal acceleration.
/ \2
=? I r- 0-223 m/s2
1224 We know from the previous problem that a satellite moving west to east at a distance R - 2*00 x 104 km from the centre of the earth will be fevolving round the earth with an angular velocity faster than the earth’s diurnal angualr velocity. Let co = angular velocity of the satellite 2л
co0 « — = anuglar velocity of the earth. Then
2л co - con « — U T
as the relative angular velocity with respect to earth. Now by Newton’s law
R2
M- 6-27 xlO24 kg
So,
Substitution gives
1225 vel°city of the satellite in the inertial space fixed frame is
east to west. With
respect to the Earth fixed frame, from the	(йГ x rf the velocity is
, 2nR /yM	.
T " n
Here M is the mass of lhe earth and T is its period of rotation about its own axis.
114
It would be -
2nR T
, if the satellite were moving from west to east.
To find the acceleration we note the formula
» F + 2m(v* xаГ) + /исо2А
HereJF» - Y R and v* 1 oTand v* x nTis directed towards the centre of the Earth.
R3
(	2
_	, yM _ 2лЛ /yM 2я /2л\ _
Thus w - i—r- + 2	_	+ V -4-	-zr -	-=-	R
R2	T “ R T I	T I
toward the earth’s rotation axis
2\T^ V R
xs
R2
2л
~f
2nR T
« 4-94 m/s2 on substitution.
1226 From the well known relationship between the velocities of a particle w.r.t a space fixed frame (K) rotating frame (K*) v*- v* i(wxr^
,	( 2 D
vi“ V- —1Я
Thus kinetic energy of the satellite in the earth’s frame
1	,21/	2лЯ\2
Ti « — mv 7- — ml v----=7—
1	2	1 2 I T I
Obviously when the satellite moves in opposite sense comared to the rotation of the Earth its velocity relative to the same frame would be
And kinetic energy
From (1) and (2)
2яЯ\2 v+ T J
/	2 л A?
\ T /
Now from Newton’s second law
у Mm mv2	- /чМ г—ъ-
or v.yy .VgJ-
(2)
(3)
(4)
Using (4) and (3) -
/ i D 2 л R \
•Try- -----------1-27 nearly (Using Appendices)
ri / 2nR\
VgR
115
U27 For a satellite in a circular orbit about any massive body, the following relation holds between kinetic, potential & total eneigy :
T--E, U-2E	(1)
Thus since total mechanical energy must decrease due to resistance of the cosmic dust, the kintetic energy will increase and the satellite will ‘fall’, We see then, by work eneigy theorm
j 2 л	аЛ dv
So,	mvdv * av vdt or, ---------- —y
Ш y2
Now from Netow’s law at an arbitray radius r from the moon’s centre.
v yM - /vM — « or v = V J— r	▼ r
(M is the mass of the moon.) Then
where K= moon’s radius. So
vf	t
dv a v2 m vx	0
°r,	----
aIvi vf) ay	aygR
vYa
where g is moon’s gravity. The averaging implied by Eq. (1) (for noncircular orbits) makes the result approximate.
1.228 From Newton’s second law
yMm mvo -ПМ	,
Jp-- — or v0- у -L- - 1-67km/s
From conservation of medianical energy
1 2_уМио 0 or>	- 2-37 km/s2
2 К	" К
(1)
(2)
116
In Eq. (1) and (2), M and R are the mass of the moon and its radius. In Eq. (1) if M and R represent the mass of the earth and its radius, then, using appendices, we can easily get
v0= 7-9 km/s and vc= 11-2 km/s.
1.229 In a parabolic orbit, E « 0
So
1
Z	J\	¥ Л
where M - mass of the Moon, R - its radius. (This is just the escape velocity.) On the other hand in orbit
2 _ yMm \/ yM mvfR=rf01 vf~ v A
Thus
Av - (1 - V2 )	-
-0-70 km/s.
1.230 From 1.228 for the Earth surface
- / yM ,	- / 2yM
vo" V к and ve- у
Thus the sought additional velocity
Av - ve - v0 -	(V2-l)-gR(V2-l)
This ‘kick’ in velocity must be given along the direction of motion of the satellite in its orbit
1.231 Let r be the sought distance, then
YW Iм 2 / D \2
——-----у - -Ц- or nr - (nR - r)
(nR-r)2 r2
or	Vn r - (nR-г) or r » 2!^ 4 - 3-8x 104 km.
1	vrj+1
1232 Between the earth and the moon, the potential energy of the spaceship will have a maximum at the point where the attractions of the earth and the moon balance each other. This maximum P.E. is approximately zero. We can also neglect the contribution of either body to the p.E. of the spaceship sufficiently near the other body. Then the minimum eneigy that must be imparted to the spaceship to cross the maximum of the P.E. is clearly (using E to denote the earth)
117
yjWgm
With this energy the spaceship will cross over the hump in the P.E. and coast down the hill of p.E. towards the moon and crashland on it. What the problem seeks is the minimum energy reguired for softlanding. That reguies the use of rockets to loving about the braking of the spaceship and since the kinetic energy of the gases ejected from the rocket will always be positive, the total energy required for softlanding is greater than that required for crashlanding. To calculate this energy we assume that the rockets are used fairly close to the moon when the spaceship has nealy attained its terminal velocity on the moon
V —— where Mo is the mass of the moon and RQ is its radius. In general
dE « vdp and since the speed of the ejected gases is not less than the speed of the rocket, and momentum transfered to the ejected gases must equal the momentum of the spaceship the energy E of the gass ejected is not less than the kinetic energy of spaceship
yMf/n
Addding the two we get the minimum work done on the ejected gases to bring about the softlanding.
/Af£ Mo\ Amn “ Ут + V
On substitution we get 1-3 x 108 kJ.
1.233	Assume first that the attraction of the earth can be neglected. Then the minimum velocity, that must be imparted to the body to escape from the Sun’s pull, is, as in 1*230, equal to
(VI-DVi
where v2 - yMs/r,r* radius of the earth’s orbit, Ms - mass of the Sun.
In the actual case near the earth, the pull of the Sun is small and does not change much over distances, which are several times the radius of the Earth. The velocity v3 in question is that which overcomes the earth’s pull with sufficient velocity to escape the Sun’s pull. Thus
Imv2- —«	\2v2
where R = radius of the earth, ME = mass of the earth.
Writing - yME /R, we get
v3» V 2 Vj + (^2 -1 )2 vi  16-6 km/s
118
1.5	DYNAMICS OF A SOLID BODY
1234 Since, motion of the rod is purely translational, net torque about the C.M. of the rod should be equal to zero.
1	/ /	\ F
Thus	F. - «	| - - a | or, тг « 1 - 77г
*2	21 2	I F2 1/2
For the translational motion of rod. F. mwc
Fj-F.-mw, „,1--.—
From (1) and (2) a	mw	2aF2
~r— - or, Z----------Im
1/2	F,	mw,
—>	—*>	—> —►
1.235 Sought moment N « rxF- (ai + bj) x (Ai + Bj)
 aB£+Ab(-E^« (aB -Ab)lc*
(1)
(2)
and arm of the force I - — - -7====== F >/A1 + BL
1236 Relative to point O, the net moment of force :
N-	+ r2 *F2 - (ai*Aj) + (BjxBi)
- abk+AB(-k)-(ab-AB)k*	(1)
Resultant of the external force
F-F^F^Ap+вГ	(2)
As N-F* 0 ( as ^±^7) so the sought arm I of the force F
1237
I- N/F-
у/а2+в*	_	_
For coplanar forces, about any point in the same plane, J ri r* Fnet
(where Fna « F,я resultant force) or, « r x F^
Thus length of the arm, I - -=,—
Here obviously | « 2F and it is directed toward right along AC. Take the origin at C. Then
about C,
N- I V2aF +	- VTaF | directed normally into the plane of figure.
(Here a = side of the square.)
Thus JV - F ~q= directed into the plane of the figure.
, F{a/yH} a	a . Atz.
I» —y	~ sin 45
2F	2V2	2
Thus the point of application of force is at the mid point of the side BC.
Hence
119
1238 (a) Consider a strip of length dx at a perpendicular distance x from the axis about which we have to find the moment of inertia of the rod. The elemental mass of the rod equals
dm " “у dx
Moment of inertia of this element about the axis
di = dmx2~ ™dx-x2
Thus, moment of inertia of the rod, as a whole about the given axis
i
Т Г m 2 J ml2 7х dx~ —
О
(b) Let us imagine the plane of plate as xy plane taking the origin at the intersection point of the sides of the plate (Fig.).
Obviously	» f dm у 2
0 х	'
m й
" 3
m i_2 Similarly
Hence from perpendicular axis theorem

which is the sought f moment of inertia.
1.239 (a) Consider an elementry disc of thickness dx. Moment of inertia of this element about the z -axis, passing through its C.M.
diz -	. p 5	< a *
2	2	U	FTTJ
where p « density of the material of the plate	*
and S « area of cross section of the plate.
Thus the sought moment of inertia	&
- jph/?4(asS- лЯ2)
120
putting all the vallues we get, Iz • 2- gm-m 2 (b) Consider an element disc of radius r and thickness dr at a distance x from the point O. Then r = x tana and volume of the disc = xx 2 tan 2 a dx
Hence, its mass dm= xx tanadx-p (where
т/|лЯ2Л)
density of the cone «
P =
Moment of inertia of this element, about the axis OA,
r2 di = dm —
L.240 (a) Let us consider a lamina of an arbitrary shape and indicate by 1,2 and 3, three axes coinciding with x, у and z - axes and the plane of lamina as x -y plane.
Now, moment of inertia of a point mass about x - axis, dlx « dm у 2
£13(3)
Thus moment of inertia of the lamina about
this axis, Ix = J* dmy2
Similarly,' Iy • Jdmx2
and Iz = f dmr 2
= fdm(x2 + y2) as r = Vx2+y2
Thus,
4 е Ix + Iy or, /3-
(b) Let us take the plane of the disc as x - у plane and origin to the centre of the disc (Fig.) From the symmetry Ix « Iy. Let us consider a ring element of radius r and thickness dr, then the moment of inertia of the ring element about the у - axis.
121
<//« dmr2~ -^j(2nrdr)r2 z	nR2
Thus the moment of inertia of the disc about z- axis
R
, 2m C 3 , mR2
1 = —z I r dr - ——
z R2J	2
0
But we have
Thus
1.241	For simplicity let us use a mathematical trick. We consider the portion of the given disc as the superposition of two* complete discs (without holes), one of positive density and radius R and other of negative density but of same magnitude and radius R/2.
As (area) a (mass), the respective masses of the considered discs are (4/n 13) and (- m 13) respectively, and these masses can be imagined to be situated at their respective centers (C.M). Let us take point О as origin and point x - axis towards right Obviously the C.M. of the shaded position of given shape lies on the x - axis. Hence the C.M. (C) of the shaded portion is given by
(-m/3 ) (-Я/2)+( 4m/3 )0 R
X'~	(-m/3) + 4m/3	" 6
Thus C.M. of the shape is at a distance R/6 from point О toward x - axis
Using parallel axis theorem and bearing in mind that the moment of inertia of a complete homogeneous disc of radius mQ and radius r0
1	2
equals — moro. The moment of inetia of the
small disc of mass (- m / 3) and radius R/2 about the axis passing through point C and perpendicular to the plane of the disc
r L(™\(r^
7C~ 2^ 3 Д 2 J Д 3 Д 2 + 6 J
mR2 4
Similarly
|гнЯ2 + ^ 3	27
Thus the sought moment of inertia,
Ic-^mR2~^jtnR2-
122
1.242	Moment of inertia of the shaded portion, about the axis passing through it’s certre,
Now, if R = r + dr, the shaded portion becomes a shell, which is the required shape to calculate the moment of inertia.
Now, / =	- — яр {( r + dr)5 - r 5}
= л p (r5+ 5r4dr +..........-r5^
Neglecting higher terms.
2 / .	2 j \ 2	2	2
» j(4rcrzdrpJr - -mr*
1.243	(a) Net force which is effective on the system (cylinder M + body m) is the weight of the body m in a uniform gravitational field, which is a constant. Thus the initial acceleration of the body m is also constant.
From the conservation of mechanical energy of the said system in the uniform field of gravity at time t - Ar : AT + AU = 0
1	2 I MR2 2 л. n
or	~mv + — —— Ц) -mgbh = 0
2	2 2	6
or,
1	2
— ( 2m + M) v - mg Ah - 0 [ as v - co/? at all times ]
(1)
But	V2- 2wAA
Hence using it in Eq. (1), we get
-- ( 2m +M)2w Ah- mg Ah = 0 or w = 4	( 2m + M )
.	................ w 2mg
From the kinematical relationship, p - — « —-77 7--
К \ 2m + M. ) К
Thus the sought angular velocity of the cylinder =	(2м+^)/?Г“ (l+M/2m)R
(b) Sought kinetic energy.
'T't л \	2 1	2	1 / л	i z \ n 2 2
ПО- 2WV +2-2-<O “ 4<2я,+л/)л m
123
1244 For equilibrium of the disc and axle 2T- mg or T- mg/2
As the disc unwinds, it has an angular acceleration P given by
Zp- 2Tr or p-
The corresponding linear acceleration is 2
rp=
Since the disc remains stationary under the combined action of this acceleration and the acceleration (-w) of the bar which is transmitted to the axle, we must have 2
1245 Let the rod be deviated through an angle q/from its initial position at an arbitrary instant of time, measured relative to the initial position in the positive direction. From the equation of the increment of the mechanical energy of the system.
AT- Aext
or,

or,
Thus,
<p
1M12	2 fr., .
— —co = I Fl coscp dtp
n
= F/sinqp
V6F simp Ml
1246 First of all, let us sketch free body diagram of each body. Since the cylinder is rotating and massive, the tension will be different in both the sections of threads. From Newton’s law in projection form for the bodies and m2 and noting that = и>2 « w = РЯ, (as
no thread slipping), we have (mx > m2) m1g-Ti^ mrw = mr&R
and T2-m2g = m2w	(1)
Now from the equation of rotational dynamics of a solid about stationary axis of rotation, i.e. Nz= IP2, for the cylinder.
or, (TX-T2)R-/p - m«2p/2	(2)
Similtaneous solution of the above equations yields :
(m1-m2)g	m1(m + 4m2)
p = —;--------г and zr = —/--1—c
T? m7(m + 4m7)
R I mr + m2 + у	2	2	1
124
1.247 As the systenr (m + тг + m2) is under constant forces, the acceleration of body /n1 an m2 is constant In addition to it the velocities and accelerations of bodies тг and ai equal in magnitude (say v and w) because the length of the thread is constant From the equation of increament of mechanical energy i.e. ДТ + ДС7 « Afr, at time t whe block m1 is distance h below from initial position corresponding to t - 0,
1 ,	, 2 1 (mR2\v2 ,	.	.
-(m1 + m2)v +-\—^~\^~m2gh--km1gh
(1
(as angular velocity co = v/R for no slipping of thread.)
But	v2»2wh
So using it in (1), we get
2 (m2 - kmr) g ----—--------Г m + 2 (+ m2)'
(2
Thus the work done by the friction force on ml
/ 1 2
A/r« -kmrgh~ -biig -wt
km^m^-km^gh2 m + 2 (+ m2)
(using 2).
1.248 In the problem, the rigid body is in translation equlibrium but there is an angular retardation. We first sketch the free body diagram of the cylinder. Obviously the friction forces, acting on the cylinder, are kinetic. From the condition of translational equlibrium for the cylinder, mg- N^kN2; N2- kNr
Hence,
*1'
mg 1+k
7! ^2
к
mg 1 + k2
For pure rotation "of the cylinder about rotation axis, Nz «
2
or, -kN1R-kN2R~
kmgR (1+k) mR2 ft
or,
2k(l + k)g (l + k2)R
Now, from the kinematical equation,
co 2 — co02 + Д <p we have,
w02 (l+k2)R
Дф « -T.-/-,—гт— , because оэ » О
4k(l+k)g
125
Непсе, the sought number of turns, Дф ^f)2 ( 1 +
П 2л 8л £ (1 + £) g
1.249 It is the moment of friction force which brings the disc to rest The force of friction is applied to each section of the disc, and since these sections lie at different distances from the axis, the moments of the forces of friction differ from section to section.
To find Nz> where z is the axis of rotation of the disc let us partition the disc into thin rings (Fig.). The force of friction acting on the considered element dfr = к (2л r dr a) g, (where a is the density of the disc) The moment of this force of friction is
dNz= -rdfr» -2nk(j gr2 dr
Integrating with respect to r from zero to Rf we get
R
Nz- - 2л к a g Jr2 dr = -jxkagR3. о
For the rotation of the disc about the stationary axis z, from the equation Nz = I$z
t d3 (nR2a)R2 Q Q 4kg nkagR = *--------or p2-
Thus from the angular kinematical equation
3R co0 4£g
1.250 According to the question,
Integrating,
or,
co =
k2t2 tf^kt
4/2 - I +“0’
(Noting that at t = 0, a) = (Dq.)
Let the flywheel stops at t» t0 then from Eq. (1), tQ =
2/7^ к
Hence sought average angular velocity
к
0
126
1.251
dMz
Let us use the equation	- Nz relative to the axis through О	I
For this purpose, let us find the angular momentum of the system Mz about the given rotation axis and the corresponding torque The angular momentum is
(1)
Mz « Jco + mvR
Я2со
m0 2
[where /» —R and v» сой (no cord slipping)]
So,
dMz (MR2 „2k
A 2 +mR
(2)
The downward pull of gravity on the overhanging part is the only external force, which exerts a torque about the z -axis, passing through О and is given by,
у xgR
1.252
Hence from the equation
dM
M^ + mR2\fi2- ^xgR
ft	/Я(М + 2т)>0
Note : We may solve this problem using conservation of mechanical energy of the system (cylinder + thread) in the uniform field of gravity.
(a) Let us indicate the forces acting on the sphere and their points of application. Choose positive direction of x and cp (rotation angle) along the incline in downward direction and in the sense of oT(for undirectional rotation) respectively. Now from equations of dynamics of rigid body i.e. F* - and Ncz - Ic we get : mg sin amw
frR - |mfl20
But	fr £ kmg cosot
In addition, the absence of slipping provides the kinematical realtionship between the accelerations : w= ₽Я	(4)
The simultaneous solution of all the four equations yields : 2	2
к cos a у sin a, or к у tan a
(b) Solving Eqs. (1) and (2) [of part (a)], we get :
Thus,
and
(1)
(2)
(3)
127
5	.
wc« - g sin a.
As the sphere starts at t« 0 along positive x axis, for pure rolling
ve(0"	| g sin at	(5)
Hence the sought kinetic energy
T~ ^mv^ + ~mR2 3u)2-	w- v/R)
7	/5	.	\2	5	2 . 2	2
- ygsinar - — mg^sin a/
1.253 (a) Let us indicate the forces and their points of application for the cylinder. Choosing the positive direction for x and <p as shown in the figure, we write the equation of motion of the cylinder axis and the equation of moments in the C.M. frame relative to that axis i.e. from equation Fx - mwc and Nz - Ic Pr
mR mg-2T- mwc-, 2TR-
As there is no slipping of thread on the cylinder
we~ pK
From these three equations
T- 13N, ₽- If- 5xl02rad/s2 o	5 К
2 e
(b) we have P - J £
2	>	2 -
So, wc » ~g > 0 or, in vector form wc » — g
3	Ж
P = F • v== F • (й£ t)
-> /2	2	2
=	-mg t
1.254 Let us depict the forces and their points of application corresponding to the cylinder attached with the elevator. Newton’s second law for solid in vector form in the frame of elevator,
gives :
2T + /ng*+ m (- w0) » /ий^	(1)
The equation of moment in the C.M. frame relative to the cylinder axis i.e. from
m/?2ft mR2 w'
2ZR=—P-—-
[as thread does not slip on the cylinder, w'» РЯ ]
QO
mWo
tvlo
128
or,
As (1) Ffl w
so in vector form
T-
mw' 4
(2)
2 > ->
Solving Eqs. (1) and (2), w « — (g - w0) and sought force
F- 2T=	(Г- iv0).
1.255 Let us depict the forces and their points of application for the spool. Choosing the positive direction for x and cp as shown in the fig., we apply Fx = mw^ and Nc^ - Ic and get
mg sin a - T = mw; Tr « /(3
“Notice that if a point of a solid in plane motion is connected with a thread, the projection of velocity vector of the solid’s point of contact along the length of the thread equals the velocity of the other end of the thread (if it is not slacked)”
Thus in our problem, vp « v0 but v0= 0, hence point P is the instantaneous centre of rotation of zero velocity for the spool. Therefore vc = cor and subsequently wc « 0r.
Solving the equations simultaneously, we get
g sin a _	.2
w = -------— = 1-6 m/s
Let us sketch the force diagram for solid cylinder and apply Newton’s second law in projection form along x and у axes (Fig.) :
1.256
frl + fr2 = mwc	(i)
and Nr + N2 - mg - F » 0
or	+ N2 = mg + F	(2)
Now choosing positive direction of <p as shown in the figure and using Ncz = Ic
we get
FR - (fr, + fr2)R=^^=^-^-	(3)
[as for pure rolling wc «	]. In addition to,
frl+fr2*k(Nl+N2)	(4)
129
and
Solving the Eqs., we get
Ikmg	_ 3kmg
(2-ЗЛ)’	““ 2-ЗЛ
Wc (max) ж к г	, к
ГП lmS + max ] ж m
m
3 kmg '
2fr?
2-ЗЛ
1.257 (a) Let us choose the positive direction of the rotation angle cp, such that w^ and P2 have identical signs (Fig.). Equation of motion, Fx = mwa and Ncz - Ic fiz gives :
F cos a - fr = mwa :frR-Fr~ Ic$z = ymR 2 ₽z
In the absence of the slipping of the spool wcx = fizR
From the three equations wcx = wc =
F [cos a - (r/R) ]
/и (1 + y)
(b) As static friction (fr) does not work on
,	r
where cos a > —
|/V
the spool, from the equation of the increment of mechanical eneigy = AT.
^mvc+^ymR2^“ jMl+Y)Vc
1	1	/1	2
— m(l+y)2wex = -m(l+y)2wcl-wct
2
p2 Icosa - —I t2 I	К j
2 m(l+y)
Note\that at cos a - r/R, there is no rolling and for cos a < r/R , wcx < 0, i.e. the spool
will move towards negative x-axis and rotate in anticlockwise sense.
1.258 For the cylinder from the equation N« I в about its stationary axis of rotation.
. _ mr n n 4T	.
2Tr= — 0 or 0- —	(1)
z	mr
For the rotation of the lower cylinder from the
equation Na - Ic 02
mr2	a, 4T a
2Tr - — 0 or, 0' = — = 0 z	mr
Now for the translational motion of lower cylinder from the Eq. Fx « mwcx :
mg-2T~ mwc	(2)
As there is no slipping of threads on the cylinders :
И>с ж p' Г + ₽r ж 2 pr	(3)
130
Simultaneous solution of (1), (2) and (3) yields
T.
10*
1.259 List us depict the forces acting on the pulley and weight A, and indicate positive direction for x and ф as shown in the figure. For the cylinder from the equation Fx» m $ and ^ez= 4₽z> We8et
Mg + TA-2T- Mwe	(1)
/w
and 2TR + TA (27?) - /0 -	(2)
For the weight A from the equation Fx- mwx
mg-TA-mwA	(3)
As there is no slipping of the threads on the pulleys.
wA » wc + 2 P R • wc + 2 wc  3 wc (4) Simultaneous solutions of above four equations gives :
3(M+3m)g
A “ / I \
- M+9m + -4
\ R )
1.260 (a) For the translational motion of the system m^)y from the equation : Fx  ти^ F~ («1 + ^)^ or, wc-F/(m1 + m2)	(1)
Now for the rotational motion of cylinder from the equation : Ncx « Ic P2
m. r2	2F
Fr= _l_p or ₽r-	(2)
z
But wK « wc + P r, So
(b) From the equation of increment of mechanical energy : AT»
Here	AT-T(r),so, T(t)-A^
As force F is constant and is directed along x-axis the sought work done.
A^ = Fx
(where x is the displacement of the point of application of the force F during time interval t)
131
/1 з\ F2 г2 (3 m,+2 mJ
F HV-------—
12 I 2 mj (mx + m^)
(using Eq. (3)
Alternate : T (t) =	(t) + Trotalu* (t)
,	x2	2 ,	.2
1 , J Ft \	1 m\ r / 2Ft\
— (m, + m0)  -------г + — —— --------- «
2 v 1	2 I (m1 + m2) I 2 2 I r I
- T(0
t2 (3	+ 2 /и2)
2	(m1+ m2)
1,261 Choosing the positive direction for x and <p as shown in Fig, let us we write the equation of motion for the sphere F « mw„ and N„ = L By
*	X	ex	CX C  X
fr“m2w2; frr-^m2r2$
(w2 is the acceleration of the C.M. of sphere.)
For the plank from the Eq. Fx « mwx
F-fr = mlwi
In addition, the condition for the absence of slipping of the sphere yields the kinematical relation between the accelerations :
M>1 » w2 + ₽ r
Simultaneous solution of the four equations yields :
F J 2 = у----—г and w2= 7 wi
1/П1 + у7П2|
1.262 (a) Let us depict the forces acting on the cylinder and their point of applications for the cylinder and indicate positive direction of x and <p as shown in the figure. From the equations for the plane motion of a solid Fx  tnwcx and Na = Ic$z'
kmg= mwcx or wcx= kg	(1)
or pz-
Let the cylinder starts pure rolling at t» f0 after releasing on the horizontal floor at t« 0. From the angular kinematical equation
or w- U}0-2^t	(3)
2V
From the equation of the linear kinematics, t ex ocx ex
or	vc = 0 + kg t0
132
But at the moment t = tQ, when pure rolling starts vc = cnA
so,
Thus
u>0R
°" 3kg
(b) As the cylinder pick, up speed till it starts rolling, the point of contact has a purely
1	2.
translatory movement equal to — wc % in the forward directions but there is also a backward
1	2
movement of the point of contact of magnitude (u>0 t0 - — £ r0) R. Because of slipping
the net displacement is backwards. The total work done is then,
A& = kmg
'о ~ (ю(/о + | ₽ФЙ
= kmg
Z	Zl К I
, M гм м
mco^2 6
The same result can also be obtained by the work-energy theorem, « A T.
1.263	Let us write the equation of motion for the centre of the sphere at the moment of breaking-off: mv2/(R + r) = mg cos 0,
where v is the velocity of the centre of the sphere at that moment, and 0 is the corresponding angle (Fig.). The velocity v can be found from the eneigy conservation law :
,	1	2	1 r 2
mgh~ -mf + - la, where I is the moment of inertia of the sphere relative to the axis passing through the sphere’s 2	2
centre, i.e. I = ~ mr . In addition,
v = cor; h = (R + r) (1 - cos 0).
From these four equations we obtain
(0= y/lOgtR + ryilr1.
1.264	Since the cylinder moves without sliding, the centre of the cylinder rotates about the point □, while passing through the common edge of lhe planes. In other words, the point О becomes the foot of the instantaneous axis of rotation of the cylinder.
It at any instant during this motion the velocity of the C.M. is vx when the angle (shown in the figure) is £, we have
m V|
—T- = mgcosfi-N,
133
where N is the normal reaction of the edge or, v2 - gR cos 0 -	(1)
From the energy conservation law, 1 v? 1 V? К	К
But A)’ ^~+mR2 ’ ^mR2’ (from the parallel axis theorem)
Thus,	Vo + |gJ?(l-cosP)	(2)
From (1) and (2) 2 gR Z« о NR v0- ^Рсозр-Д) - —
The angle p in this equation is clearly smaller than or equal to a so putting p = a we get vo= ^-(7 cos a-4) -
where No is the corresponding reaction. Note that 7/ NQ. No jumping occurs during this turning if NQ > 0. Hence, v0 must be less than
Vmax-	(7 cos a - 4)
1265 Clearly the tendency of bouncing of the hoop will be maximum when the small body A, will be at the highest point of the hoop during its rolling motion. Let the velocity of C.M. of the hoop equal v at this position. The static friction does no work on the hoop, so from conservation of mechanical energy; Er - E2
Zv\2	\2
or, 0 +	+	-mgR* (2v)2 + v2 + ^mR2 + mgR
or,	3v2-v2-2gfl	(1)
From the equation Fn - mwn for body A at final position 2 :
2
mg+N’ = тио)2Я= m || R	(2)
|л J
134
As the hoop has no acceleration in vertical direction, so for the hoop,
(3)
From Eqs. (2) and (3), жг _ mv2 N-2mg~ —	(4)
As the hoop does not bounce, N i 0	(5)
So front Eqs. (1), (4) and (5),
SgR-v^	,
—i 0 or 8gR*
Hence	v0£ V8 gR
1.266	Since the lower part of the belt is in contact with the rigid floor, velocity of this part becomes zero. The crawler moves with velocity v, hence the velocity of upper part of the belt becomes 2v by the rolling condition and kinetic energy of upper part 1 f m\ 2	2
= 2 T @v) = mv ’ wbich is also the sought kinetic eneigy, assuming that the length of the belt is much larger than the radius of the wheels.
1.267	The sphere has two types of motion, one is the rotation about its own axis and the other is motion in a circle of radius R, Hence the sought kinetic energy
+ (1)
where is the moment of inertia about its own axis, and I2 is the moment of inertia about the vertical axis, passing through (9,
2	2	2	2	2
But, Л ж уmr and 4 “ 5 mr + (using parallel axis theorem,)	(2)
In addition to
V	V
- — and co, » —	(3)
1 r 2 R	v 7
7 I \
Using (2) and (3) in (1), we get T' » — mv2 11 + ^~2j
1.268 For a point mass of mass dm, looked at from C rotating frame, the equation is dm w' - f + dm co2 r* + 2 dm (v* x w)
where r* » radius vector in the rotating frame with respect to rotation axis and
v = velocity in the same frame. The total centrifugal force is clearly
Fcy« dmco2/- mw2Rc —>
Rc is the radius vector of the C.M. of the body with respect to rotation axis, also
F = 2mv xw cor	c
where we have used the deflnitions
mRc = ^dmr* and mv*c - dmv*
135
1269 Consider a small element of length dr at a distance x from the point C, which is rotating in a circle of radius r  x sin 0
Now, mass of the element = ^yj dx
So, centrifugal force acting on this element
(fn\	7
- — dr co x sin 0 and moment of this force
О1
about С,
drco2xsin0-xcos0
yy~ sin 2 0 x2 dx
А
and hence, total moment 1/2
N = 2 I sin 2 0 x2dr« 777 m co2 /2 sin 2 0, J 21	24
о
1.270 Let us consider the system in a frame rotating with the rod. In this frame, the rod is at >
rest and experiences not only the gravitational force m g and the reaction force R, but also the centrifugal force Fcf.
In the considered frame, from the condition of equilibrium i.e. NQz = 0 Ncf = mg | sinG
where Ncf is the moment of centrifugal force about O. To calculate Ncf , let us consider an element of length dr, situated at a distance x from the point O. This element is subjected to । /лЛ 2 a horizontal pseudo force у dr co xsin0.
The moment of this pseudo force about the axis of rotation through the point О is /	л
dNcj*= у I dx co x sin 0x cos 0
or,
I
m co2 . n n 2 , —j— sin 0 cos 0 x dx
I
/mco2 . n n о, ma)212
—j— sin 0 cos 0 xr dx о
It follows from Eqs. (1) and (2) that,
So
cos 0
ZZZ/1ZZZZ (?i
sin 0 cos 0
or 0 = cos ”1 ( —
2 co2/
(i)
F
(2)
(3)
136
1.271
1.272
When the cube is given an initial velocity on the table in some direction (as shown) it acquires an angular momentum about an axis on the table perpendicular to the initial velocity and (say) just below the C.G.. This angular momentum will disappear when the cube stops and this can only by due to a torque. Frictional forces cannot do this by themselves because they act in the plain containing the axis. But if the force of normal reaction act eccentrically (as shown), their torque can bring about the vanishing of the angular momentum. We can calculate the distance Дх between the point of application of the normal reaction and the C.G. of the cube as follows. Take the moment about C.G. of all the forces. This must vanish because the cube does not turn or tumble on the table. Then if the force of friction is fr
fr |= N Дх
But N = mg and fr = kmg, so
Дх = ka/2
In the process of motion of the given system the kinetic energy and the angular momentum relative to rotation axis do not vary. Hence, it follows that
l Ml2	2	1	Z	2,2	,2,	1ЛА2	2
—= —m( co I +v ) + —co 2 3	0	2	v	7	2 3
ДХ
Initial angular momentum
Initial velocity
A)cis± to the initial velocity on the table
and
(a> is the final angular velocity of the rod) Ml2 Ml2 ,2
From these equations we obtain
’о
and
1.273
y' = w0 / / Vl + Зт/M
Due to hitting of the ball, the angular impulse received by the rod about the C.M. is equal to p i If (o is the angular velocity acquired by the rod, we have
mZ2 pl	6p
— ш-^-orw-^	(1)
In the frame of C.M., the rod is rotating about an axis passing through its mid point with the angular velocity co. Hence the force exerted by one half on the other = mass of one half x acceleration of C.M. of that part, in the frame of C.M.
m ( 2 I \	9p2
- — co — - m-т—» 9N
2	4 I 8 2ml
137
1274 (a) In the process of motion of the given system the kinetic energy and the angular momentum relative to rotation axis do not vary. Hence it follows that
1	2	1	,2 l(Ml2\ 2
-mv = -mv + - —— to 2	2	2	3
and
l , I Ml2 mv — ** mv — + —— cd 2	2	3
From these equations we obtain , (Зт- 4M \	.	4v
Зт + 4Af J	Z (1 + 4 m/3M)
As v t t v, so m vector form v » -------— v
I	Зт + 4M I
(b) Obviously the sought force provides the centripetal acceleration to the C.M. of the rod and is
Frt = mwcn
„ г I 8Mv2
2	I (1 + 4M/2>m )2
1.275 (a) About the axis of rotation of the rod, the angular momentum of the system is conserved. Thus if the velocity of the flying bullet is v.
. ( ,2 Ml2\
mvl - \ml + -y~ I co
—«г;-«г as ” " " m + T z
Now from the conservation of mechanical energy of-the system (rod with bullet) in the uniform field of gravity
|(mi2 + ^j-jco2- (Af+m)g|(l-cosa)	(2)
' /
[because C.M. of rod raises by the height — (1 - cosa ) ]
Solving (1) and (2), we get
zn ( cdZ ) + Af I cd — I -mv
(b) Sought Ap -
where.cd/ is the veloccity of the bullet and cd — equals the velocity of C.M. of the rod
after the impact. Putting the value of v and co we get
This is caused by the reaction at the hinge on the upper end.
138
(с)
Let the rod starts swinging with angular velocity co', in this case. Then, like part (a)
( Ml2 2I , mvx = ~з~^тх Iю
or co' -
3mvx
Ml2
Final momentum is
So, This vanishes for
A	/ 3*
bp-Pf-Pi- "”'1 -^--1
3
1.276 (a) As force F on the body is radial so its angular momentum about the axis becomes zero and the angular momentum of the system about the given axis is conserved. Thus
1
M
(b) From the equation of the increment of the mechanical energy of the system :
MR2	„2 MR2
—-— co0 + m со0л = —-— co or co = co0
1 MR2 2 1 (MR2 22®	2
+ m R 2 соц = A,
Putting the value of co from part (a) and solving we get
2m
2	I’’ .И
1.277 (a) Let z be the rotation axis of disc and qp be its rotation angle in accordance with right-hand screw rule (Fig.). (<p and qp' are to be measured in the same sense algebraically.) As Mz of the system (disc + man) is conserved and Mу« 0, we have at any instant,
or,
On integrating
<p	<₽'
fd<p= ~f( ;(т/2)Рф/
•'I	+ v 1^2' ) I
0	O'	'
or,
(1)
This gives the total angle of rotation of the disc.
139
(b) From Eq. (1) dtp dt
dt
Differentiating with respect to time
dt2
1 dv'(t)
R dt
W1 +v \ z /
Thus the sought force moment from the Eq. Nz = 10 m0R2 / m1 1 m2 R \ z /
A = ----------x_e _ -—
z 2 dt2 2
dt
Hence
=	dv'(t)
z Ъп1 + m2 dt


R


1.278 (a) Frome the law of conservation of angular momentum of the system relative to vertical axis z, it follows that:
/1ю1г + /2<о2г- (Il+I2)a>z
Hence	co2- (/1(оь + /2Ю2г)/(Л+/2)	(1)
Not that for u)z > 0, the corresponding vector ю coincides with the poitive direction to the z axis, and vice versa. As both discs rotates about the same vertical axis z, thus in vector form.
(О- /1a>1+/2a>2/(/1+/2)
However, the problem makes sense only if co1 ft (02 or f 1 co2
(b) From the equation of increment of mechanical energy of a system: Ajr = AT.
Using Eq. (1)
A	hh f	\2
Af'~ 2(1^12)^и Ю2г)
1.279	For the closed system (disc + rod), the angular momentum is conserved about any axis. Thus from the conservation of angular momentum of the system about the rotation axis of rod passing through its C.M. gives :
I mv- = mv
l тптг — +	—co
2	12
(1)
140
(у' is the final velocity of the disc and co angular velocity of the rod) For the closed system linear momentum is also conserved. Hence
mv = mv' + T] mvt
(2)
(where vc is the velocity of C.M. of the rod)
From Eqs (1) and (2) we get /co	,
vc = — and v - v « T)vc
Applying conservation of kinetic energy, as the collision is elastic
1	2	1	,2 1	2 1 wnl1 2 * * * * 2
-mxr = -mv + rW; + —	- co (3)
2	2	2 1 c 2 12 v 7
v2 - v'2 = 4r)Vc2 and hence v + v' = 4vc
or
Then
L
, 4-T) j 12 v
v = -----L v and co » 7-----—
4 + T)	(4 + Y|) I
Vectoria 11 y, noting that we have taken v parallel to v
v
и
So, и « 0 for Y) « 4 and и | f v for т] > 4
1.280	See the\ diagram in the book (Fig. 1.72)
(a)	When the shaft BB' is turned through 90° the platform must start turning with angular velocity Q so that the angular momentum remains constant. Here
A) <*>o
(Z+/o)£2-/0coo or, Й= 2®.
1 +2o
The work performed by the motor is therefore
1 о 1 A?
L(I+T 1O2 = — —______
2U	2I + I0
If the shaft is turned through 180°, angular velocity of the sphere changes sign. Thus from conservation of angular momentum,
/ Q - A) % “ A) %
(Here -Z0co0 is the complete angular momentum of the sphere i. e. we assume that the angular velocity of the sphere is just - co0). Then
co0
Q=2f0-^
and the work done must be, l_rO2 Xr 2 X r 2 _ ^A) Mo
Z Q + 2 A)	2 A)	” j
141
(b) In the case (a), first part, the angular momentum vector of the sphere is precessing with angular velocity Q. Thus a torque, л2 2
*o %
/0 (o0 Q = -—г- is needed.
2 +/q
1.281 The total centrifugal force can be calculated by,
I y-(o2xdx = — m l0 co2 о /0	2
Then for equilibrium, I lo
and, T2 + T\ = m l0 co 2
Thus T\ vanishes, when
l0
Then T2== mg j = 25N
0
1.282 See the diagram in the book (Fig. 1.71).
(a) The angular velocity co about OO' can be resolved into a component parallel to the rod and a component co sin0 perpendicular to the rod through C. The component parallel
to the rod does not contribute so the angular momentum
1	2
M » I co sin0 = — m I co sin0 12
Also,
Mz = Msin0 = ^m/2cosin20
This can be obtained directly also,
—>
(b) The modulus of M does not change but the modulus of the change of M is | A M |. | AM | - 2Msin (90 - 0) = ^m/2cosin20
(c) Here = M cos0 = I cd sin0 cos0
T n n	1	,2	2 . 2л
= I co sin0 cos0 —3— = — ml cd sin 0 dt 24
Now
dM dt
as M precesses with angular velocity cd.
142
1.283 Here M = Zco is along the symmetry axis. It has two components, the part ZcocosO is constant and the part M± - Zco sinG presesses, then
dM di
= Z co sin0 co' = mgl sin0
or,
co'  precession frequency 
mgl Zco
0-7jad/s
(b)	This force is the centripetal force due to precession. It acts inward and has the magnitude
|F| - |S т‘ “'2 Pi| = mco'2Zsin0 = 12 mN.
is the distance of the i th element from the axis. This is the force that the table will exert on the top. See the diagram in the answer sheet
1.284 See the diagram in the book (Fig. 1.73).
1	2
The moment of inertia of the disc about its symmentry axis is —mR . If the angulai
1	2
velocity of the disc is co then the angular momentum is — m R co. The precession frequency being 2л и,
we have
dM i p2 о
—- = — mR co x 2лп
dt 2
This must equal m (g + w) Z, the effective gravitational torques (g being replaced by g + w in the elevator). Thus, co -	= 300 rad/s
лйи
143
1285
1.286
g + w inclined at angle tan" ~ with the vertical. Then with reference to the new " vertical” we proceed as in problem 1*283. Thus
m/Vg2 +w2 ш	= Q.g ra(j/s>
7co
The vector co forms an angle 0 - tan” ~ « 6° with the normal vertical.
2	2
The moment of inertia of the sphere is ^mR and hence the value of angular momentum
2	2
is - mR co. Since it precesses at speed co the torque required is
2 a
“ mR co co' = F' I
So,
F’~ jmR2wm'/l - 300N
(The force F ' must be vertical.)
1287
1	2	1	2
The moment of inertia is — mr and angular momentum is — mr co. The axle oscillates about a horizontal axis making an instantaneous angle.
, 2л/
<p= <pmsm —
This means that there is a variable precession with a rate of precession The maximum 2лфт
value of this is ——. When the angle between the axle and the axis is at its maximum value, a torque Zco Q
1288
1	2
= — mr co —— = ------------- acts on it.
л mr2(ocpm
The corresponding gyroscopic force will be--—-----= 90 N
The revolutions per minute of the flywheel being л, the angular momentum of the flywheel v
is I x 2лл. The rate of precession is —
Thus# = 2nINV/R - 5*97 kN. m.
1289
As in the previous problem a couple 2nInv/R must come in play. This can be done if a force, acts on the rails in opposite directions in addition to the centrifugal and other Rl forces. The force on the outer rail is increased and that on the inner rail decreased.
The additional force in this case has the magnitude 1*4 kN. m.
144
1.6
1.290
ELASTIC DEFORMATIONS OF A SOLID BODY
But
Variation of length with temperature is given by
lt« lQ (1 + aAr) or у x a А/ « e zo
и e“
Thus a - aAtE, which is the sought stress of pressure.
Putting the value of a and E from Appendix and taking Ar = 100°C, we get a  2-2 x 103 atm.
1.291
1.292
(1)
(a) Consider a transverse section of the tube and concentrate on an element which subtends an angle A<p at the centre. The forces acting on a portion of length AZ on the element are
(1) tensile forces side ways of magnitude G&r&l. The resultant of these is
2crArAZsin aArAZAqp
radially towards the cente.
(2) The force due to fluid pressure - ргАфА/
Ar
Since these balance, we get	am—
'	с?	л max m
where am is the maximum tensile force.
Putting the values we get pmax « 19-7 atmos.
(b) Consider an element of area dS - л ( г AO/2 )2 about z -axis chosen arbitrarily. There are tangential tensile forces all around the ring of the cap. Their resultant is
I\ ( A о 2л г — Ar
Hence in the limit
P„H m
. AO sm —
Ar де
2 a Ar or = ------------- - 39-5 atmos.
Let us consider an element of rod at a distance x from its rotation axis (Fig.). From Newton’s second law in projection form directed towards the rotation axis
-dT~ (dm)w2x- yo)2xdk
On integrating
2 2 /ИО) X Л ,	v
- T - —-—— + C ( constant)
145
But at
Thus
Hence
x = ± or free end, T = 0
_ ими212 „	„ mw2l
0 -	— + C or C -
_ mw2 ( l_ x2^
1= 2	4" I
Гтах ’ (at mid P°“0 О
Condition required for the problem is ^max” $ c mw 2l c	2
So, —------Som or w- y
Hence the sought number of r p s
Thus
co 1 n = — _ —
2л л/
[using the table n = 0*8 x 102rps ]
1293 Let us consider an element of the ring (Fig.). From Newton’s law Fn « mwn for this element, we get,
dQ ) a)2r [see solution of 1.93or 1.92}
I 2л I
So,
T- ^~co2r 2л
Condition for the problem is :
T	т(л)2г
—x s;	or, —y—x-s
nr2 m 2л2 r2
2 2n2oMr or, (O_s '«	ж «5
лг2(2лгр) pr2 Thus sought number of rps
Пж 2л 2лг
Using the table of appendices n • 23rps
1.294 Let the point О desend by the distance x (Fig.). From the condition of equilibrium of point O.
2TsinO« mg or T=
2sin0
2
(1)
Now,
T	d
--------7 o« e£ or T eE л — n(d/2)2	4
( о here is stress and e is strain.)
(2)
146
In addition to it,
V(//2)2+x2
From Eqs. (1), (2) and (3)
1.295 Let us consider an element of the rod at a distance x from the free end (Fig.). For the considered element *T-T* are internal restoring forces which produce elongation and dT provides the acceleration to the element. For the element from Newton’s law :
dT - (dm)w - (ydx\— - ~rdx
I I I m I
As free end has zero tension, on integrating the above expression,
p 5»	p
I dT J dx or T-~x
Elongation in the considered element of lenght dx :
d£-y(x) dx-^dx-L	ML
SEL
Fo f	Fol
Thus total elengation |»--- I xdx = --- -
SEI J	2SE
Hence the sought strain
I~2SE
1.296 Let us consider an element of the rod at a distance r from it’s rotation axis. As the element rotates in a horizontal circle of radius r, we have from Newton’s second law in projection form directed toward the axis of rotation :
T-(T+d7)- (dm)<x?r
or,	-dT^ dr) to2 r - у co2 r dr
147
At the free end tension becomes zero. Integrating the above experession we get, thus о	/
Thus
-J dT^ т
m<n2(l2-r2
2 = —-— —-—
m 2 Г j yco J rdr
.2
Elongation in elemental length dr is given by : Л 5-	° (r) j ' T ,
l	dzi
(where S is the cross sectional area of the rod and considered element) m co21 /	r2\.
Thus the sought elongation
T is the tension in the rod at the
fc Cah mw21 d^~-2ST
I (1-4^г
/1 i2
0 '	'
, m cd2 I 21
1 О (D2 Z3
« —	— (where p is the density of the copper.)
3 E
3SE
1.297 Volume of a solid cylinder
V « mr2Z
So,
AV я2гArZ яг2AZ _ 2Ar AZ
V itr2! +	r 1
(1)
But longitudinal strain AZ/Z and accompanying lateral strain A r/r are related as
Ar AZ
—--ну
(2)
Using (2) in (1), we get :
V’f (1-2и)
(3)
But
А/ -f/nr2 I “ E
(Because the increment in the length of cylinder AZ is negative)
So,
2
148
Thus,
-Fl ^v~~- (l-2p) lh
Negative sign means that the volume of the cylinder has decreased.
1.298 (a) As free end has zero tension, thus the tension in the rod at a vestical distance у from its lower end
T- ”gy	(1)
Let dl be the elongation of the element of length dy, then
dl - ^dy
= -^=dy =	= pgydy/E (where p is the density of the copper)
SE SIE
Thus the sought elongation
i
Ы-/ dl- Pgf^~ \pgl2/E	(2)
0
(b) If the longitudinal (tensile) strain is e «	, the accompanying lateral (compressive)
strain is given by	, Ar e - — - - pe r	(3)
Then since V « я r21 we have
AV 2Ar А/ V “ r + I
-(l-2p)y [Using (3)]
where ~~ is given in part (а), ц is the Poisson ratio for copper.
1.299 Consider a cube of unit length before pressure is applied. The pressure acts on each face. The pressures on the opposite faces constitute a tensile stress producing longitudianl com* pression and lateral extension. The compressions is £ and the lateral extension is p
E	E
The net result is a compression
(1 - 2p) in each side. E
„ AV 3p „ . v	AV AZ
Hence — = - у- (1 - 2 p) because from symmetry — = 3 —
149
(b) Let us consider a cube under an equal compressive stress a, acting on all its faces.
Then,	volume strain =	(1)
where k is the bulk modulus of elasticity. ~	О 3 O _ V
So	Г'£’(1-2и)
or,	E- 3*(1-2ц)“ |(l-2p)(as k~
p \	“/
Ц “ if £ and p are both to remain positive.
1300 A beam clamped at one end and supporting an applied load at the free end is called a cantilever. The theory of cantilevers is discussed in advanced text book on mechanics. The key result is that elastic forces in the beam generate a couple, whose moment, called the moment of resistances, balances the external bending moment due to weight of the beam, load etc. The moment of resistance, also called internal bending moment (I.B.M) is given by
I.B.M. = EI/R
Here R is the radius of curvature of the beam at the representative point (x, у). I is called the geometrical moment of inertia
I.pdS
of the cross section relative to the axis passing through the netural layer which remains unstretched. (Fig.l.). The section of the beam beyond P exerts the bending moment A^(x) and we have,
El XTf X R ‘
If there is no load other than that due to the weight of the beam, then
*W- ^pg(l-x)2bh where p = density of steel. Hence, at x = 0
(I\ pgl2bh
Wo' 2EI
	
	
J	N
	
150
1301
We use the equation given above and use the result that when у is small
ThUS R dx2 ’ dx2 El
(a)	Here N (x)  No is a constant. Then integration gives, dy No*r dx~ El 1
But
0 for x - 0, so С\ « 0. Integrating again,
N0x2
У“ 2EI
where we have used у « 0 for x « 0 to set the constant of integration at zero. This is the equation of a parabola. The sag of the free end is
X-y(x- 0-
L £ 1
(b)	In this case N (x) = F(l- x) because the load F at the extremity is balanced by a similar force at F directed upward and they constitute a couple. Then
d2y F(l-x)
El •
Integrating,
dy_ F(lx-x2/2) dx El 1
As before Cr = 0. Integrating again, using у - 0 for x « 0
F\2	6/	Fl3
У--------й— here к- —
Here for a square cross section
a/2
- a4/12.
-л/2
1302
One can think of it as analogous to the previous case but with a beam of length 1/2 loaded upward by a force F/2.
Thus
48 E7’
On using the last result of the previous problem.
1303
1	2
(a)	In this case N (x) = — pg b h (I - x) where b = width of the girder.
Also
b h3/12. Then,
161
Ebh2 d2y pgbh
П dx2' 2
(l2-2lx+x?).
Integrating, using
6£&62x_&2 + ^ dx E h2 \	3)
- 0 for x* 0. Again integrating
W/V-Z/
У Eh2 \ 2	3	12j
Thus
x 6pg/4zi 1 г
Eh2 2 3 + 12
6 Pg/4 3 PS*4 Eh2 12	2 Eh2
d2v
(b) As before, El —£ - N (x) where N (x) is the bending moment due to section PB. dx
This bending moment is clearly
21
N~ I wtZ^-x)-wZ(2Z-x)
w I212 - 2x1 + у - wl (21 -x) - w
(Here w = p g b h is weight of the beam per unit length)
dy	(X	11
Now integrating, El w I — - -^-1 + c0
Jy	з
or since , = 0 for x « Z, cn = wl /3 dx	u
Integrating again, EIy= w
wl3 x
3 +C1
As у = 0 for x » 0, cx» 0. From this we find
.	.	_	5wZ4/_r 5 pg/4
y(x- Z)-	El- re> x
7	24 2ЕЛ2
1304 The deflection of the plate can be noticed by going to a co- rotating frame. In this frame each element of the plate experiences a pseudo force proportional to its mass. These forces have a moment which constitutes the bending moment of the problem. To calculate this moment we note that the acceleration of an element at a distance § from the axis is a  § P and the moment of the forces exerted by the section between x and Z is
152
N- plhflf |р/Лр(/3-х3). X
From the fundamental equation
El4j}“ |р/Лр(/3-х3). cur
+ h/2 C 2 lh3 The moment of inertia I- I z Idz^ —-J	12.
-Л/2
Note that the neutral surface (i.e. the surface which contains lines which are neither stretched nor compressed) is a vertical plane here and z is perpendicular to it.
^~2 ”	(/3-*3)- Integrating
dx Ehz
Ф = 4PP(/3.r ^|C
dx E h2 \	4 J 1
Since	= 0, for x = 0, Cj» 0. Integrating again,
4 p P fl3x2 x5^
У~ TF[~2O} + C2
c2 - 0 because у - 0 for x « 0
Thus	k= y(x= Z)=
1305 (a) Consider a hollow cylinder of length Z, outer radius r + Ar inner radius r, fixed at one end and twisted at the other by means of a couple of moment N. The angular displacement qp, at a distance I from the fixed end, is proportional to both I and N. Consider an element of length dx at the twisted end. It is moved by an angle qp as shown. A vertical section is also shown and the twisting of the parallelopipe of length I and area Ar dx under the action of the twisting couple can be discussed by elementary means. If f is the tangential force generated then shearing stress is f/Ar dx and this must equal
G 0 « G , since 0 «
Hence,	f - GArdr^.
The force f has moment f r about the axis and so the total moment is
N-
153
(b)	For a solid cylinder we must integrate over r. Thus r
V2
1.306 Clearly N - J' l^Aj^pG = G <p (d24 -d/2
using	G = 81 G Pa = 8-1 x IO10 Д-
m
d2 = 5 x 10 ~ 2 m, dx = 3 x 10 ~ 2 m
cp = 2-0° = radians, I = 3 m
N = (625 - 81) x 102 N‘m X О X
= 0-5033 x 103 N-m « 0-5 к N-m
1.307 The maximum power that can be transmitted by means of a shaft rotating about its axis is clearly N co where N is the moment of the couple producing the maximum permissible torsion, (p. Thus
_ л; r4 Gqp
P « —- -1 • co = 16*9 kw
1308 Consider an elementary ring of width dr at a distant r from the axis. The part outside dN
exerts a couple N + — dr on this ring while the part inside exerts a couple N in the
opposite direction. We have for equilibrium
dN, м
-—dr= -dlti dr
154
where dl is the moment of inertia of the elementary ring, p is the angular acceleration and minus sign is needed because the couple N (r) decreases, with distance vanshing at the outer radius, N (r2) » 0. Now
Thus
or,
dl« ---—5- 2л r dr r2
2m P 3 j
—о—^r dr
fr?--?)
KT 1 m P / 4	4\
N - —	~ r '> on mtegratlon

1309 We assume that the deformation is wholly due to external load, neglecting the effect of the weight of the rod (see next problem). Then a well known formula says,
elastic energy per unit volume
77 stress x strain - 77 a e
2	2
™ .	1 m „ 2
This gives — —E e
« 0-04 kJ for the total deformation energy.
1310 When a rod is deformed by its own weight the stress increases as one moves up, the stretching force being the weight of the portion below the element considered.
The stress on the element dx is
pл^(/-^g/rcr2« pg(l-x)
The extension of the element is
Adx = d&x = pg(l-x)dx/E 1	2
Integrating AZ» — p g l /Е is the extension of
the whole rod. The elastic energy of the element is
I pg(l-x) ?8<!-x)
Integrating
AI7= |nr2 p2g2Z3/E- j-n^ZE^y
155
1.311 The work done to make a loop out of a steel band appears as the elastic energy of the loop and may be calculated from the same.
If the length of the band is 4 the radius of the loop R = Now consider an element 2 я
ABCD of the loop. The elastic energy of this element can be calculated by the same sort of arguments as used to derive the formula for internal bending moment. Consider a fibre at a distance z from the neutral surface PQ. This fibre experiences a forcep and undergoes ds Z
an extension ds where ds « Z d qp, while PQ « 5 « Я J qp. Thus strain — = — If a is the cross sectional area of the fibre, the elastic energy associated with it is
1 F(z\2
Rdy a
Summing over all the fibres we get
,	•	1 я2 E h 63 n no i т
So the energy is	—-----------= 0-08 kJ
1.312 When the rod is twisted through an angle 0, a couple
^(0)“
Л Cz
——— 0 appears to resist this. Work done in twisting the rod by an angle qp is
then
<₽
JJV(0)d0- ^£<p2 = 7J
0
on putting the values.
1.313
л r^ dr
The energy between radii r and r + dr is, tiy differentiation, —— G qp
Its density is
nr3 dr G<p2 _ 1 G<p2r2 2itrdrl I = 2 i2
1.314 The energy density is as usual 1/2 stress x strain. Stress is the pressure pg/i. Strain is p x p gh by defination of p. Thus
1	?	4
и = — P (p gh) = 23-5 kJ/m on putting the values.
166
1.7
HYDRODYNAMICS
1.315
Between 1 and 2 fluid particles are in nearly circular motion and therefore have centripetal acceleration. The force for this acceleration, like for any other situation in an ideal fluid, can only come from the pressure variation along the line joining 1 and 2. This requires that pressure at 1 should be greater than the pressure at 2 i.e.
Pi>P2
so that the fluid particles can have required acceleration. If there is no turbulence, the motion can be taken as irrotational. Then by considering
along the circuit shown we infer that
1316
v2 V1
(The portion of the circuit near 1 and 2 are streamlines while the other two arms are at right angle to streamlines)
In an incompressible liquid we also have div v « 0
By electrostatic analogy we then find that the density of streamlines is proportional to the velocity at that point.
From the conservation of mass ж V2^2
But Sj < S2 as shown in the figure of the problem, therefore
vi > v2
As every streamline is horizontal between 1 & 2, Bemoull’s theorem becomes 1	2
p + — p v - constant, which gives
(1)
Pi < p2 as Vi > v2
As the difference in height of the water column is ДА, therefore p2 -Pl - pgM
(2)
From Bemoull’s theorem between points 1 and 2 of a streamline 1	2	1	2
>1 + 2 p vi “ ^2 + 2 pv2
P2 “ Pl “ | P (V1 " V2> | P (v? -
or,
pgAA -
using (1) in (3), we get
or
A / 2gA/t V1 “ S2 V s2 - s*
Hence the sought volume of water flowing per see
(3) (using Eq. 2)
d2 -
157
1.317
Applying Bernoulli’s theorem for the point A and B, Pa= Pb + ^Pv2 as> va “ 0
or. |pv2“ Рл~Рв“ длРо£
-/2AAp0g
So,	v = V------
P
Thus, rate of flow of gas, Q = S v =
n/2A/»p0g
V P
The gas flows over the tube past it at B. But at A the gas becomes stationary as the gas will move into the tube which already contains gas.
n 1 2
In applying Bernoulli’s theorem we should remember that — + — v + gz is constant along P 2
a streamline. In the present case, we are really applying Bernoulli’s theorem somewhat indirectly. The streamline at A is not the streamline at B. Nevertheless the result is correct. To be convinced of this, we need only apply Bemoull’s theorem to the streamline that goes through A by comparing the situation at A with that above В on the same level. In steady conditions, this agrees with the result derived because there cannot be a transverse pressure differential.
1.318 Since, the density of water is greater than that of kerosene oil, it will collect at the bottom. Now, pressure due to water level equals hr px g and pressure due to kerosene oil level equals p2 g. So, net pressure becomes px g + h2 p2 g.
From Bernoulli’s theorem, this pressure energy will be converted into kinetic energy while flowing through the whole A.
i.e. Aj Pi g + Л2 p2 g = | pi v2
Hence v »
3 m/s
1.319 Let, H be the total height of water column and the hole is made at a height h from the
bottom.
Then from Bernoulli’s theorem |pv2- (H - h) pg
or, v = V (H - h) 2g, which is directed horizontally. For the horizontal range, I» v t
>/2g(H-ti)	= 2 V(HA - A2)
8
158
Now, for maximum Z,
d(g/»-/>2) _ dh
which yields	h « — « 25 cm.
1320 Let the velocity of the water jet, near the orifice be v', then applying Bemoullis theorem, 1	2 u 1	2
2PV я *oP£ + 2Pv
or,	v' - >/v2-2gh0	(1)
Here the pressure term on both sides is the same and equal to atmospheric pressure. (In the problem book Fig. should be more clear.)
Now, if it rises upto a height h, then at this height, whole of its kinetic* energy will be converted into potential eneigy. So, 1 2pv'2- pgh or Л- —
v2
- 5— Ao “ 20 cm, [using Eq. (1)]
1.321 Water flows through the small clearance into the orifice. Let d be the clearance. Then from the equation of continuity
(2jtrd)v« (2nR^Z)v2
or v1A1=vr=v27?2	(1)
where , v2 and v are respectively the inward radial velocities of the fluid at 1, 2 and 3. Now by Bernoulli’s theorem just before 2 and just after it in the clearance
t 2
Po + hPg-P2+ 2pv2	(2)
Applying the same theorem at 3 and 1 we find that this also equals
1	2 I 2	/o\
P + -pv = p0+ -pV!	(3)

(since the pressure in the orifice is p0 )
From Eqs. (2) and (3) we also hence
<2gh
and
1	2
P “ Po + 2 pV1
= Po + hPS 1
[Using (1) and (4)]
169
1311 IxX faxct otl pisWbe F and Xhe tengfh of the cylinder be I. Then, work done - Fl	(1)
theorem for points 1	2
A and B,p» “pv where p is the density and v is the velocity at point B. Now, force on the piston, F-pA- |pv2A
where A is the cross section area of piston. Also, discharge through the orifice during time interval t« Svt and this is equal to the volume of the cylinder, i.e.,
(2)
у
V- Svt or — D*
(3)
From Eq. (1), (2) and (3) work done
- Ip^A/-I pA-^I-JpV3/S3r2 (as A/-V)
2r	2 K (st)2	'	7
1323 Let at any moment of time, water level in the vessel be H then speed of'flow of water through the orifice, at that moment will be
v- y/2gH In the time interval dt, the volume of water ejected through orifice, JV- svdt
On the other hand, the volume of water in the vessel at time t equals
V- SH
Differentiating (3) with respect to time, ~ S^- or dV- SdH dt dt
(1)
(2)
(4)
Eqs. (2) and (4) SdH* svdt or dt
S dH
—== .from (2)
sN2gH
о
S
Integrating,
।	J	-	.	dh
I	dt-	—t=	I	~r=
J	s'JlhJ	УН
0	h
Thus,
1324 In a rotating frame (with constant angular velocity) the Eulerian equation is
-Vp + pg*+ 2p(v* х(о5 + ро)2Г=р
In the frame of rotating tube the liquid in the "column* is practically static because the orifice is sufficiently small. Thus the Eulerian Eq. in projection form along r* (which is
160
the position vector of an arbitrary liquid element of lenth dr relative to the rotation axis)
reduces to
+ pO)2r»0 dr
or, dp = p<n2 rdr p	r
so, J dp = pco2 J rdr
p0	- Ю
Thus
P 0 - PQ +
Hence the pressure at the end В just before the orifice i.e.
/W-Л + ^(2/Л - A2)
Then applying Bemoull’s theorem at the orifice for the points just inside and outside of the end В
p0 + i p co2 (2 / A - A2) = p0 + у p v2 ( where v is the sought velocity) 2	2t
1.325 The Euler’s equation is P^“ f-^P’ - V (p + pgz), where z is vertically upwards.
Now	+	C1)
at dt
But	(v^ V) Vv2j - vx Curl v*	(2)
we consider the steady (i.e. dv^dt« 0) flow of an incompressible fluid then p « constant.
• and as the motion is irrotational Curl v^» 0
So from (1) and (2)	pV^|-p2j*-V(p + pgz)
—1	2	\
or,	V p + -pv +pgz - 0
1	2
Hence	p + - p v + P S2 ж constant.
1326 Let the velocity of water, flowing through A be vA and that through В be v# then discharging rate through A « QA“ SvA and similarly through В - Svg.
Now, force of reaction at A,
fa~ pQava~ PSv%
161
Непсе, the net force, pS(y2-v%) as	(1)
Applying Bernoulli’s theorem to the liquid flowing out of A we get
Po + pgh - Po + J PVA and similarly at В
1	2 S
Po + Р«(Л+ЛЛ) - po + у pvB
2	В
Hence	(vb - vA) = A/ipg
Thus	F = 2pgShh - 0-50 N
1327 Consider an element of height dy at a distance у from the top. The velocity of the fluid coming out of the element is
v~ y/2gy
The force of reaction dF due to this is dF« p dA v2, as in the previous problem, = p(bdy)2gy h
Integrating	F = p gb J* 2y dy
h-1
= pgb[h2-(h-1)2 ] = pgbl(2h-l)
(The slit runs from a depth h -1 to a depth h from the top.)
1328 Let the velocity of water flowing through the tube at a certain instant of time be u, Zhen
Q	2
и « where Q is the rate of flow of water and я r is the cross section area of the tube, яг
From impulse momentum theorem, for the stream of water striking the tube comer, in x-direction in the time interval dt, Fxdt~ - pQudt or » - pQu and similarly, Fy « p Q и
Therefore, the force exerted on the water stream by the tube,
F- -pQui + pQuj
According to third law, the reaction forcj on the tube’s wall by the stream equals (-F)
- pQui-pQuj.
Hence, the sought moment of force about 0 becomes
N « I (- i) x (p Qu ip Qu j') « p Qu I к -
and	1^1 “
nr
0-70 N-m
162
1329 Suppose the radius at A is R and it decreases uniformaly to r at В where S - nR2 and 5 « лг. Assume also that the semi vectical angle at 0 is a. Then
К ж г ж 2.
£2 Lr х
So	у - г + R~r (х - L1}
Ь2-Ь1
where у is the radius at the point P distant x from the vertex O, Suppose the velocity with which the liquid flows out is V at A, v at В and и at P. Then by the equation of continuity
лК2У « n:r2v * лу2и
The velocity v of efflux is given by
v
and Bernoulli’s theorem gives
1	2	1	2
V 2 pW “ P° + 2 PV/
where pp is the pressure at P and p0 is the atmospheric pressure which is the pressure just outside of B. The force on the nozzle tending to pull it out is then
F = J (Pp-Po) sin0 ^yds
We have subtracted p0 which is the force due to atmosphenic pressure the factor sin 0 gives horizontal component of the force and ds is the length of the element of nozzle surface, ds - dx sec 0 and
ten0 -
L2 hl
Thus
F - J* | (v2 - и2) p 2лу dx h	2
JR
« яр I V2 (1 - у dy Jr \ У )
2 1 (n2 2	2^ i ( 1t(R2 - Г2)2
- л p v - A - r + —= - r « p gh \ —— 2	R2 )	[ R2 )
« pgh (S - s)2/S « 6-02 N on putting the values.
Note : If we try to calculate F from the momentum change of the liquid flowing out wi will be wrong even as regards the sign of the force.
There is of course the effect of pressure at S and 5 but quantitative derivation of F fron Newton’s law is difficult.
163
1330 The Euler’s equation is p —- f~Vp Ле space fixed frame where f « -pgk downward. We assume incompressible fluid so p is constant.
Then f = - V (pgz) where z is the height vertically upwards from some fixed origin. We go to rotating frame where the equation becomes
p~~-« - V (p + pgz) + p co2 r +2p (v* xw)
the additional terms on the right are the well known coriolis and centrifugal forces. In the frame rotating with the liquid v « 0 so
V^p + p£Z-|p(oV)= 0
or	p + pgz-^-pco2^» constant
On the free surface p  constant, thus ft)2 2 z~ — r + constant
If we choose lhe origin at point r « 0 (i.e. the axis) of the free surface then “cosntant” = 0 and ft)2 2
z « — r (The paraboloid of revolution)
At the bottom z = constant
So	p = — p co2 r2 + constant
If p = pQ on the axis at the bottom, then
P- Po + |p“2r2-
1331 When the disc rotates the fuild in contact with, corotates but the fluid in contact with the walls of the cavity does not rotate. A velocity gradient is then set up leading to viscous forces. At a distance r from the axis the linear velocity is 0) r so there is a velocity gradient
both in the upper and lower clearance. The corresponding force on the element whose h
radial width is dr is
T] 2jtrdr — (from the formular F = rjA — )
The torque due to this force is
r) 2nzJr	r
h find the net torque considering both the upper and lower clearance is n
2 J i]2 dr — о
« ^R4anr\/h
So power developed is
P « лЛ4со2т|/Л « 9-05 W (on putting the values).
(As instructed end effects i.e. rotation of fluid in the clearance r > R has been neglected.)
164
1332 Let us consider a coaxial cylinder of radius r and thickness dr, then force of friction or dv
viscous force on this elemental layer, F» 2itrli\ —.
This force must be constant from layer to layer so that steady motion may be possible.
or,	« 2 л I T| dv.	(1)
Integrating,
„ I dr - . Г .
Fl — = 2л I n I dv
Jr	J
or, F In I ~-j = 2л I t| v	(2)
\ 2/
Putting	r = Rr, we get
Ri
F In — - 2 л I Y| v0
From (2) by (3) we get,
In r/R2 v=
Note : The force F is supplied by the agency which tries to carry the inner cylinder with velocity v0 .
1333 (a) Let us consider an elemental cylinder of radius r and thickness dr then from Newton’s formula
, duj _	,	2 do
F = 2 л rl n r — = 2 л I Y| r — 'dr	'dr
and moment of this force acting on the element,
N= 2лг2/т]~г= 2nr3/”qy-dr	dr
or, 2 л I r| dm « N —
(2)
As in the previous problem N is constant when conditions are steady
<0
Integrating,	2 л It] J'da)- N
0 Ъ
or,
(3)
Putting	r » <o “ <o2 > we 8et
1 1
2 Л IT] o>2 “
2
(1)
165
From (3) and (4),

J_ _ г Я2'г2
(b) From Eq. (4),
w N .
Ni~ J- 4лт]<о2
R21R22
r2-r2
1334 (a) Let dV be the volume flowing per second through the cylindrical shell of thickness
dr then,
dV = - (2 л r dr) v0
and the total volume,
R
(b)	Let, dE be the kinetic energy, within the above cylindrical shell. Then dT= |(dm)v2- |(2nr/drp)v2 1	•	_ /	«2
2r3 r5L
Я2 Я4
Hence, total energy of the fluid, R
(c)	Here frictional force is the shearing force on the tube, exerted by the fluid, which
equals - r| 5 —.
Given,
So,
And at
dv ~ r
2 v0 —у
dr 0 R2
dv 2 vo r’R’ dT-~R
166
(dv\
—I
ar Jr - R
-2лЯт] I
4 л т) v01
(d)	Taking a cylindrical shell of thickness dr and radius r viscous force, -т](2лг0^,
Let tsp be the pressure difference, then net force on the element = Дрлг2 + 2лг]/г^ But, since the flow is steady, « 0
(y \ 2V° R21 or, lSp~ -------------5----« ----------5---------« 4r)v0Z/R2
яг	nr
1.335 The loss of pressure head in travelling a distance I is seen from the middle section to be h2 - hY - 10 cm. Since h2 - Ax « h1 in our problem and Л3 -	« 15 cm « 5 + Zi2 -	,
we see that a pressure head of 5 cm remains incompensated and must be converted into kinetic energy, the liquid flowing out. Thus
2
= pg A h where A h « h3 -
Thus	v« У 2gtsh  1 m/s
1.336 We know that, Reynold’s number (Re) is defined as, Re * p v Z/т), where v is the velocity I is the characteristic length and r] the coefficient of viscosity. In the case of circular cross section the chracteristic length is the diameter of cross-section J, and v is taken as average velocity of flow of liquid.
P^i V1
Now, Re (Reynold’s number at xx from the pipe end) -	 where vx is the velocity
at distance xx
p d2 v2	Ret	dr vx
and similarly,	R* « -------- so - —----------
П	\	d2v2
From equation of continuity, Ax vx « A2 v2 or,	ЛГ2ГХ= ЛГ2У2 or dXVxrx- ^2У2г2

Thus
еаЛх- 5
1.337 We know that Reynold’s number for turbulent flow is greater than that on laminar flow?
XT	/D \ Pvd 2PlVlrl j /ох ^92^2
Now, (Ke),=	——— and (Ke)t-    
167
But, (АД ь (АД Pi viri Л2
so v2 ------------- ж 5 ц m/s on putting the values.
v pod
1338 We have R - —-— and v is given by
бЛТ]ГР- -^(p-po)#
(p • density of lead, p0 = density of glycerine.)
^(P-Po^-i^tP-Po)^
Thus	j’ 77-2(P-Po)£Po<*3
z 18 T]
and	d = [9 т]2/Ро (P ” Po) g J173 ® 5*2 mm on putting the values.
1339	rn— - л^-6яг]гу
dv 6 л Ti r or	— +------—v« g
dt m 6
dv r ,	6 я Ti r
or	—+ fcv« g, Jt- ----*—
dt 9 m
todv , и h d ь to
or	e —r- + ke v- ge or -=-e v- ge
dt	6 dt b
or veh-|eto + C or v-j + Ce“te (where C is const.)
Since	v- 0 for Г 0, 0 *	+ C
к
So	c “ ~ f
к
Thus	v= 1(1-6'^)
The steady state velocity is v differs from ?• by n where n к or	r« —Inn
к 4Л r3P
Th	<	_ 4r2P_
US	к 6 л г] r 18 т] 18 т]
We have neglected buoyancy in olive oil.
168
1.8 RELATIVISTIC MECHANICS
1.340 From the formula for length contraction
So,	1 - ^2 « (1 - T))2 or v « c Vrj (2 - T])
1.341 (a) In the frame in which the triangle is at rest the space coordinates of the vertices are
(V 3 ci \ / v3 ci ।
a	, 01 I a	, 01, all measured at the same time t. In the moving
frame the corresponding coordinates at time t' are
A : « 0,0), В : (| V3 Vl - p2 + vt', 0 The perimeter P is then
and C:(^V3 Vl - p2 + w',-£,0
2	1	2
P= a + 2a^|(l-p2) + ^ = a(l +V4-302 j
(b) The coordinates in the first frame are shown at time t. The coordinates in the moving frame are,
The perimeter P is then
P= aVl - p2 + |[1 -P2 + 3]1/2x 2» a(Vl-p2 +V4~p2 ) here P= у
1.342 In the rest frame, the coordinates of the ends of the rod in terms of proper length /0
A : (0,0,0) В : (Zo cos0o, lQ sin0o , 0)
at time t. In the laboratory frame the coordinates at time t' are
A : (vr',0,0),B : |/ocos0o V 1 - ₽2 + vf, /osin0o,O
169
Therefore we can write,
I cos 0O « Zo cosOq Vl - 02 and I sin 0 « Zo sin0o
Hence /2- (/2)(ffls2e + (l- p2.)±inl8
4	1 - ₽
VI - P2sin2G~
1 - p2	Д ^_J---------------------------
1343 In the frame К in which the cone is at rest the coordinates of A are (0,0,0) and of В are (Л, h tan 0,0). In the frame X7, which is moving with velocity v along the axis of the cone, the coordinates of A and В at time r' are
A : (- vt', 0,0), В: (h Vl - p2 - vf, h tan 0, o) Thus the taper angle in the frame К is f o, tan 0 ( У'в-У'а\ tan 0 « “t== - —-----------------—I
_ p2 \ XB~xa) and the lateral surface area is, S « л h'2 sec0' tanO'
- л h2 (1 - P2) >an9 V1+^~4 - 50V1-P2cos2e
* 1 - p2 1" 0
Here So « л Л2 secO tanO is the lateral surface area in the rest frame and
Л'- Л Vl - p2 , p- v/c.
1344 Because of time dilation, a moving clock reads less time. We write,
Thus,	t-At- zVl-p2 , P- , J 2 Ar / Ar\	э 1- r + r “ 1-₽
or,	«/At/- АЛ v- с V— 2-— ’ t 1 t 1
1345 In the frame К the length I of the rod is related to the time of flight Al by Z« vAr
In the reference frame fixed to the rod (frame X')the proper length Zo of the rod is
given by	/0« vAz'
But	>1 и и OO. <4 > 1 тЧ И n OQ. 1 1
170
Thus,
So
A,, AT v Ar * -- .
. . ч2	Г- t —
i o2 /Ar\	лЛ /ArV
!-P - ы or v-cV1-fc
and
1.346
l0 - с V(Az')2-(At)2 - c ZU'
The distance travelled iq the laboratory frame of reference is vA t where v is the velocity of the particle. But by time dilation
ZU- -j=== So v- cVl-(Af(/ZU)2
VI - v2/c2
Thus the distance traversed is
cA/Vl -(AZj/A/)2
1.347 (a) If t0 is the proper life time of the muon the life time in the moving frame is t0	vt0
-=. —and hence /- —7==
Vl-vW	Vl-v2/c2
Thus	t0- ~V1 - v2/c2
U V
(The words "from the muon’s stand point" are not part of any standard terminology)
1348 In the frame К in which the particles are at rest, their positions are A and В whose coordinates may be taken as, A : (0,0,0),В = (Zo,O,O)
In the frame K' with respect to which К is moving with a velocity v the coordinates of A and В at time t' in the moving frame are
A - (yt', 0,0) В - L Vl-P2 + vt', o.oY ₽ -
Suppose В hits a stationary target in К after time t'B while A hits it after time tB + Ar. Then,
/0Vi-₽2+W'B- v(x'b + ZU)
So,
. v Ar
° Vl-v2/c2
1349 In the reference frame fixed to the ruler the rod is moving with a velocity v and suffers Lorentz contraction. If Zo is the proper length of the rod, its measured length will be
Arx- /OV1 -₽2 , p- 1
171
In the reference frame fixed to the rod the ruler suffers Lorentz contraction and we must have
Ax2 Vl - ₽2 - l0 thus Zo - V/Ar1Ar2
and
l-p2-
or V- c
Дхх
1350 The coordinates of the ends of the rods in the frame fixed to the left rod are shown.
The points В and D coincides when
/о- q-vfo or t0~ —у
The points A and E coincide when
< /	7	ci + A) Vl - B:
o - C1 +10 Vi - p2 - vtl, h	—£
From this
2c2At//0 2/д/Аг
V“ l + ^Az2//2" 1 + (lf/с hi)2
1351 In Kq, the rest frame of the particles, the events corresponding to the decay of the particles are,
A: (0,0,0,0) and (040,0)» В
In the reference frame Kf the corresponding coordintes arc by Lorentz transformation
A : (0,0,0,0), В:
' vlp C2V1-P2
vT^p2"
Now
by Lorentz Fitzgerald contraction formula. Thus the time lag of the decay time of В is vlo	vl vl
'c2VT7^2(1-p2)’c2-v2
В decays later (B is the forward particle in the direction of motion)
1352 (a) In the reference frame К with respect to which the rod is moving with velocity v, the coordinates of A and В are
A : t, xA + v (t - tA ), 0,0
В: f, xB + v (r - tB), 0,0
172
Thus I - xA - xB - v (tA - tB) - l0 Vl-p2
„	. XA-XB-V(tA-tB)
So L  --------—=--------
Vl -Л'с2
(Ь) * ^0 ” v 0л ~ *в) “ ж A1	c
(since xA - xB can be either + /0 or - Zo.)
Thus v (tA - tB) = (± 1 - V1-v2/c2) lo
1353 At the instant the picture is taken the coordintes of A,B,A',B' in the rest frame of AB are
A: (0,0,0,0)	Д/	gf
В : (0, /о, 0,0)	0=====O--------------> V
В': (0,0,0,0)	П	---
________ А	В
А':((>!-/OV1 -v2/c2 ,0,0)
In this frame the coordinates of B’ at other times arc Bf: (r, vt, 0, 0). So B' is opposite to
/0
В at time t (B)« In the frame in which B', A' is at rest the time corresponding this
is by Lorentz tranformation.
-----------(--41- -Vl-v2/c2 ^r~7\v c v Vi A c
Similarly in the rest frame of A, B, te coordinates of A at other times are
( л/	1
A : IГ, - Zo V 1 “ ~2 + 0> 01 \	c	f
lo Л.1 7"
A' is opposite tu A at time t (A) * — у 1 -
The corresponding time in the frame in which A', B' are at rest is zo
Г(А')- Y»(A)- 7
1354
By Lorentz transformation f «
173
So at time
Г- 0, r' -
vx 1
c2 Vl - v2/c2
Ifx > 0 f < 0, if x < 0, t' > 0 and we get the diagram given below "in terms of theX-clock".
К: ©0ФФФФФ
*' ФФФФФФФ
The situation in terms of the Kf clock is reversed.
1355 Suppose x (r) is the locus of points in the frame К at which the readings of the clocks of both reference system are permanently identical, then by Lorentz transformation
t' =
1	( Vx(t)\_
Vl-V2/c2 ( c2 J
So differentiating x (r) «
V c
Let	p « tan AO, Os 0 < <» , Then
c К 1	cos АО Л 1 \
x(r)« ---— (1-Vl-tanA 0 « c . : - 1 ----------—
v 7 tan A0 \	/ sm h 0 I	cos A 01
cos h 0 - 1	- /cos h 0 - 1	x , 0
- c —;——— « с V-------——- « c tan h — s v
sm h 0 v cos h 0 + 1	2
• (tan A 0 is a monotonically increasing function of 0)
1356 We can take the coordinates of the two events to be
A : (0,0,0, 0) В : (Дг, л, 0, 0) Ы
For В to be the effect and A to be cause we must have At > . c
In the moving frame the coordinates of A and В become
\a
we must have Ar' >
c
174
1357 (a) The four-dimensional interval between A and В (assuming Ay = Az « 0) is : 52-32 = 16 units
1358
1359
Therefore the time interval between these two events in the reference frame in which the events occurred at the same place is ^16 = 4m
4	4 о
or	t R- t д « — « — x 10 ~ s
B A c 3
(b) The four dimensional interval between
A and C is (assuming Ay = Az « 0)
32 - 52 » - 16
So the distance between the two events in the frai in which they are simultaneous is 4 units = 4m.
By the velocity addition formula vx-V v Vl - V2/c2 v* *4 ’V y i—г	i--V
c	c
and
,	ra-^2 У(ух-У)2 + у?(1-У2/с2)
V' - Vv'x + V y--«----------------------
1 — x.2
(a)	By definition the velocity of apporach is dxx dx2
^approach" df - dF" V1 - С" V2> " V1 + V2
in the reference frame К .
(b)	The relative velocity is obtained by the transformation law vl*v2
V1 V2 1 + x.2
V1 -	v2>
,	V1("V2)
1------5---
• v =
1360
The velocity of one of the rods in the reference frame fixed to the other rod is TZ v + v 2v
1+?
The length of the moving rod in this frame is
, fVi
1361 The approach velocity is defined by
-> dr. dr0	.
у ж ___________£ ___± e у _ V
approach dt dt 12
V2	2
V1 + v2
176
On the other hand, the relative velocity can be obtained by using the velocity addition formula and has the components
1.362
The components of the velocity of the unstable particle in the frame К are
I ч / V2 I \	C f
so the velocity relative to К is
V ?
The life time in this frame dilates to and the distance traversed is
Vv2 + y'2-(y'2 V2)/c2
Vl-V2/c2 Vl-v'2/c2
1363 In the frame K! the components of the velocity of the particle are
, v cos 0 - V
V x v V cos 0
1------Z5---
, vsinOVl - V2/c2
V as -------------
У	V V
1-—ycosO c
tt ♦ л/ vy vsmO .Г7ЛTZ2A / 2 Hence, tanO = ~r = -----——V(l-V )/c
’	v'x vcosO-V v f
1.364 In К the coordinates of A and В are
A : (f, 0, - v't', 0); В: (t1,1, - v't', 0)
After performing Lorentz transformation to the frame К we get A:t= у f В it - у
x» yVt’ y(/+ Vt') у» v' tf y^-v't'
z= 0	0
VI
By translating t' -* t'-we can write
c
the coordinates of В as В : t = у f
©
—
-->x
176
1.365
In К the velocities at time t and t + dt are respectively v and v + wdt along x - axis which
—>
is parallel to the vector V. In the frame K' moving with velocity V with respect to K, the
velocities are respectively,
v - V . v + wdt-V ----^and ------------v
1 —T	1 - (v + w dt) -y
c2	c
The latter velocity is written as
v-V
wdt v -V wV.
---V-------
1 — V —у 1 — V —у I 1 —	Z“|
C cl c I
Also by Lorentz transformation
1t dt-Vdx/c2 , 1-vV/c2 dt' « —=====« dt —=====
Vl - V2/c2 Vl - V2/c2
Thus the acceleration in the K' frame is
(b) In the К frame the velocities of the particle at the time t and t + di are repectively
(0, v, 0) and (0, v + wdt, 0) —>
where V is along x-axis. In the К frame the velocities are
(- V,vVl-V2/c2 ,o)
and	(- V, (v + wdt) Vl - V2/c2 , ()) respectively
177
Thus the acceleration , wdrV(l - V2/?)	(	,
w = -------—--------• w 11 - —I along the y-axis.
We have used dt1 = -7=== Vl-V/c2
1366 In the instantaneous rest frame v « V and и>' = ----------------------------(from 1365a)
fl-^1
S°’ ~ 1--\ c/
wf is constant by assumption. Thus integration gives w’t
S/l + №
* I c I
1367
Integrating once again x -
The boost time t0 in the reference frame fixed to the rocket is related to the time т elapsed
1368
on the earth by
We define the density p in the frame К in such a way that p dx dy dz is the rest mass dmQ of the element That is p dx dy dz - p0 dxQ dyQ dzQ , where p0 is the proper density
1369
dx0, dyQ, dzQ are the dimensions of the element in the rest frame Kq. Now
dy» dy0> dz» dzQ, dx» dx0
178
if the frame К is moving with velocity, v relative to the frame KQ. Thus
or
1.370 We have
or
Defining T] by p = p0 (1 + q)
П(2 + П) (1+П)2
So —-c
. x2
1 I mo C 1 x 100 % Ш 4 -M x 100 %
Ц p )
1.371 By definition of T), mov	v2	1
— - r)»»ov or i" —w ~2 T	c	n
1.372 The work done is equal to change in kinetic energy which is different in the two cases Classically i.e. in nonrelativistic mechanics, the change in kinetic energy is
1 m0 с2 {(0-8)2 - (0-6)2 ) - | m0 c2 0-28 - 0-14 m0 c2
Relativistically it is, 2	2
moc	mQc
Vl-(0-8)2 Vl - (0-6)2
2	2
m0 c moc 0-6 " 0-8
m0c2 (1-666 -1-250)
= 0-416 m0c2= 0-42 moc2
178
1374 Relativistically
So
Thus
But Classically, 0t/- д/-^ so	i-Z—= e
mQc	Pd	4 mQc
T 4
Hence if	----- < т E
moc 3
the velocity 0 is given by the classical formula with an error less than £.
1375 From the formula
Vl-v2/c2
we find or
rr2 2 ?2	2 4	/	2 тл2 2 2	2 4
xi - c p + i?Iq c or (^o c — P
T(2m0c2 + 7)= c2p2 i.c. p= |vT(2m0c2 + T)
1376 Let the total force exerted by the beam on the target surface be F and the power liberated there be P. Then, using the result of the previous problem we see
F~ Np- ^у/т(Т+2т0с2) - £ VT(T+2m0c2)
since I« Nef N being the number of particles striking the target per second. Also,
These will be, respectively, equal to the pressure and power developed per unit area of the target if I is current density.
180
1377 In the frame fixed to the sphere The momentum transferred to the eastically scatterred particle is
2mv
The density of the moving element is, from 1.369, n —
'Ч
and the momentum transferred per unit time per unit area is ,	2mv	1	2mnv2
p = the pressure = ---n —   - —  • v = -z~
/2	« V
Vi--
cL	c2	C
In the frame fixed to the gas When the sphere hits a stationary particle, the latter recoils with a velocity
v +v
2v
1+7 m'2v 7^77
The momentum transferred is
2mv
. 4,	. 2mv
and the pressure is-«-
1-A
• л • v =
4v2/c2 (l-v2/c2)2 2mnv2
1378
v= 0 for 0
or
Fct y/(m0c)2 + (Ft)2

Fct 4*—_ £ |	----. £л/f2 ? + c2 + constant
y/F2t2 + n^c2 FJ y/f + (moc)2 F
or using x - 0 at t - 0, we get, x
2 2 {m0c2\2
'• *— -T-
181
1379
?~t2 , so x« v =
,2
.2
2t2
or,
V

c21
—  Thus a
d dt
mov
V1-^
\ C
/
1380
d ( dt
mov
-4
“ m0
V
л2 mQ c
a
V —
— + m0 -z v • v c
1	2
1
хЛ S
Thus
7*	W	_
mo	, и>« V, wLv
vi-p2
m° (l^F2’ is
1381
By definition, r	c2
E= m0—=
vx i-d
mQ c3 dt	v,
Px-rnQ
cmodx ds
where ds2 - c2 dt2 - dx2 is the invariant interval (dy = dz- 0) , dr'	(dx-Vdt) Px-VE/c2
px“ cmn~r~ cmot -------J--- “ / -t-f
x ° ds ° ds Vi _ v2/c2
Thus,
/ VdA dt--f „ idt' 3	\ c ) E-VP>
E " moc ~dTC ----&
1382
c
For a photon moving in the x direction
cpx,Py~ Рг~ 0,
1	(	ч /1— V/c
In the moving frame, e' «	- V -j = e у ।
1383
Note that
As before
or ₽- I
V‘T-
' e г 1
e ’ 2 lf’ 4
3dt	dx
E~moc
182
Similarly
dy	dz
py-m*cTs'm°c
Then Ё2 - c2p2 • E2-c2 (p2 +pj+ p2^
2 4 (C2 dt2 - dx2 - dy2 - dz2)	2 4. .	. „
* mQc —--------^ —2...'--------“ moc 1S invariant
1384 (b) & (a) In the CM frame, the total momentum is zero, Thus
Z. cpb У/Т(Т + 2т0с2) _ ./ т c ^1+^2" T + 2m0c2	* T + 2moc2
where wc have used the result of probiem (1.375) Then
1 1  у/T+2m0 c~
Vl - V2/c2 - /	т	Ъп0
VI------£---2
T + 2m0 c
 Total energy in the CM frame is
.	= 2m0c2ж V2m0с2(T + 2zn0с2) = T + 2m0c2
y/\-V2/c2	2m0c2
So
T= 2moc2
Also 2 ’ c2 p2 + hiq c4 » V2m0 с2 (T + 2zn0 c2) , 4 c2^2 » 2m0 с2 T, or p » ^m0T
i-385 Afoc2 = 4e2-c2P2
^(2m0c2 + T)2- T(2m0 c2 + T) = V2m0 c2 (2m0 c2 + 7) = c V2m0 (2m0c2 +7)
. ............ 2	/ T
Also cp= NT(T+2mac2) , v= eV---------------z-
F	0	E V T+2m0c2
1386 Let T - kinetic eneigy of a proton striking another stationary particle of the same rest mass. Then, combined kinetic eneigy in the CM frame
- 2т0с2(^1+—-11- 2Г, (-Ц+1] - 1+-^—J ' 2m0c / lmoc ) 2m0c
T' _T(2moc2 + T) 2Т(Т+2тос2) *	.«I
183
1387 We have
Ei + E2 + £3“ moc2> Р1+Я+Рз“ 0
Hence	(m0c2 -EJ2 - c2p^ - (E2 + £3)2 - j2 c2
The L.H.S.	= (/Wq c4 - Er)2 - c2p| = (m% + m2) c4 - 2m0 c2 Et
The R.H.S. is an invariant We can evaluate it in any frame. Choose the CM frame of the particles 2 and 3.
In this frame R.H.S. = (E'2+E'3)2 = (m2-i m3)2 c4
Thus (/Wq + m2) c4 - 2m0 c2 EY « (m2 + m3)2 c4
~ m <2 г I m2 a. ™2	(™	**• \21 z-4 л, г	+ wi ~ (m2 + тз) 2
I	i	2 mQ
1388 The velocity of ejected gases is и realtive to the rocket. In an earth centred frame it is
v - и
1-™ c
in the direction of the rocket The momentum conservation equation then reads
(m + dm) (y + dv)+ V (- dm) = mv
or
0
Here - dm is the mass of the ejected gases, so
mv2
~U+ c2	(	v2\
= о or + i/ l--r dm= 0
i ffv	I	c2
c2
(neglecting 1 - — since и is non- relativistic.)
Integrating
1+P	♦ *
-—Б- + — In m « constant 1-p c
The constant = — In mQ since [3=0 initially.
Thus
PART TWO
THERMODYNAMICS AND MOLECULAR PHYSICS
2.1 EQUATION OF THE GAS STATE • PROCESSES
2.1 Let Wj and m2 be the masses of the gas in the vessel before and after the gas is released Hence mass of the gas released,
Д/и - m1-m2
Now from ideal gas equation
PlV~ ml^To andP2V= m2 T0
as V and T are same before and after the release of the gas.
so,	(Pi-p2) v= (m1- m2)^TQ= \m^T0
or,
ispVM RT0 “ RT0
(1)
Am «
We also know p « p — T so, -	= —
Af RT0 p0
(where pQ » standard atmospheric pressure and TQ = 273 K)
From Eqs. (1) and (2) we get
Am = pV-^= 1-ЗхЗОх^Д= 30g Po	1
(2)
2.2 Let be the mass of the gas enclosed.
Then,	Pi V « Vi R 7\
When heated, some gas, passes into the evacuated vessel till pressure difference becomes Ap. Let p\ and p'2 be the pressure on the two sides of the valve. Then p\ V= v^R T2 and
p'2 V’ V>2R T2 ’ (V1 - v'l) R T2
185
But,
So,
or,
, v (piv	, (pi AK
р2У (я Ту R T2 j 01 P 2 “	” T2 j T2
p'l-p'l" bp
, (pi Л+Ук
jP2=	T2 ) 2
P1P2 ,	.
• -7— -P2~^P
21
,	1 (Pl T2	\
nJ ж -	- Др . 0-08 atm
r2 2 Г	M
2.3 Let the mixture contain vx and v2 moles of ff2 and He respectively. If molecular weights of H2 and Hc are My and Л/2, then respective masses in the mixture are equal to
= vt My and m2 = v2 M2
Therefore, for the total mass of the mixture we get, m » my + m2 or m » vx My + v2 M2	(1)
Also, if v is the total number of moles of the mixture in the vessels, then we know, v=Vl + v2	(2)
Solving (1) and (2) for and v2, we get,
(v M2 - m)	m- ^'My
V1" M2-My ’ v2 M2-My
(v M2 - m)	(m-v Mr)
Therefore, we get m. « Мл • -----—— and	—-----——
1	1 M2-MY 2	2 M2-Mr
mi	(v M2 - m)
or	— e-----------
’	m2 M2 (m-vMy)
One can also express the above result in terms of the effective molecular weight M of the mixture, defined as, m	RT
M-—=m—~ v	pV
my My M2-M 1-M/M2
ThUS>	m/* И2 M-My" M/My-1
Using the data and table, we get :
M~ 3-0 g and, — = 0-50 m2
186
2.4 We know, for the mixture, N2 and CO2 (being regarded as ideal gases, their mixture too behaves like an ideal gas)
p V = v R T, so pQ V = vRT
where, v is the total number of moles of the gases (mixture) present and V is the volume of the vessel. If and v2 are number of moles of N2 and CO2 respectively present in the mixture, then
v « Vi + v2
Now number of moles of N2 and CO2 is, by definition, given by
m2
= — and> v2 e 77"
where, тг is the mass of N2 (Moleculer weight = in the mixture and m2 is the mass of CO2 (Molecular weight = ЛЛ) in the mixture.
Therefore density of the mixture is given by
mY + m2 m1 + m2
P= V ° (v7?77P0)
pQ тг + т2 pQ(m1 + m2)M1M2
RT vt + v2 RT M2 + m2
«1-5 kg/m3 on substitution
23 (a) The mixture contains , v2 and v3 moles of O2, N2 and CO2 respectively. Then the total number of moles of the mixture
v = vi + v2 + v3
We know, ideal gas equation for the mixture tz n	vRT
pV=vRT or p = ............
(y<+v2 + v3)RT
or,	p « ----------« 1-968 atm on substitution
(b) Mass of oxygen (<92) present in the mixture :
Mass of nitrogen (V2) present in the mixture : m2 = v2 M2
Mass of carbon dioxide (CO2) present in the mixture : m3 = v3 M3 So, mass of the mixture
m = m1 + m2 + m3 = Mr + v2 M2 + v3 M3
Moleculer mass of the mixture : M =
mass of the mixture total number of moles
vx + v2M2 + v3 M3
Vi + v2 + v3
36-7 g/mol. on substitution
187
2.6 Let pY and p2 be the pressure in the upper and lower part of the cylinder respectively at temperature T& At the equilibrium position for the piston :
pr S + mg « p2 S or, pr +	« p2 (m is the mass of the piston.)
So,
(1)
Hence,
ят0
But pr - —— (where Vo is the initial volume of the lower part) П 4)
mg RT0 mg RTo( 1\ nV s ~ v0 or’ s' v0 ( nJ
Let 7* be the sought temperature and at this temperature the volume of the lower part becomes V, then according to the problem the volume of the upper part becomes tf V
"К. Ml' S V I n'
(2)
From (1) and (2).
П ЯГ / JP
Vo nJ V <
or,
T I 1 — I
20 I T)J
I П,
As, the total volume must be constant,
V0(l+n)- Г(1+л')
or,
v.
(i + n')
Putting the value of V in Eq. (3), we get
т 1i _ —-I v
r° 1 n °(1+л')
V0(l-^ к
T0(n2-l)n'
-V-----— = 0-42 к К
(n'2-i)n
2.7	Let p2 be the density after the first stroke. The the mass remains constant
VP-(V+AV)P1, or, P1 =
Similarly, if p2 is the density after second stroke
/ у \	/ у \2
VP1- (V+AV)P2 or, P2- I—^dPi- IvVavI Po
In this way after nth stroke.
( V \n Pn~ iV+Avl Po
Since pressure a density,
188
Рп V+ДУ Ро
(because temperature is constant)
Pn	1
It is required by — to be ~ Po	П
so,
Hence
2.8	From the ideal gas equation p - — —
dp RT dm	...
dT mvU	(1)
In each stroke, volume v of the gas is ejected, where v is given by
V г	1
v= —Г - mN\ mN[ N 1 "J
In case of continuous ejection, if corresponds to mass of gas in the vessel at time
Z, then mN is the mass at time t + Az, where Az, is the time in which volume v of the gas v
has come out. The rate of evacuation is therefore — i.e.
Az
C-	m (z + Az) - m(t)
At m(t + Az) Az
In the limit Az - 0, we get
From (1) and (2) dp	C mRT	C	dp	C	,
dt	V MV	Vp	p	V
т	I dp C I ,	. p C
Integrating I = - — I dt or In « - — t
J	P VJ	Po v
p	1
Thus	p = pQe~Ctlv
2.9 Let p be the instantaneous density, then instantaneous mass = Vp. In a short interval dt the volume is increased by Cdt.
So,	’	= (V+Cdz)(p + Jp)
(because mass remains constant in a short interval dt)
189
dp	С .
so,	-~dt
P	V
с-	. dp C J
Since pressure a density « - — dt
Рг
Pi
V	Pi	V 1
or	r = — In —« —In — 1-0 min
C	p2 C	n
2.10 The physical system consists of one mole of gas confined in the smooth vertical tube. Let zn1 and m2 be the masses of upper and lower pistons and and S2 are their respective areas.
For the lower piston pS2 + m2g- p0S2 + T, or, T" (p-p0)S2 + m2g Similarly for the upper piston PoSl + T+mlg’‘ PS1> or, T- (p-p^Si-n^g From (1) and (2) (P-Po)(S1-S2)“	+
or> *P-Po>kS=mg
so, p = ~7^+Ро~ constant До
From the gas law, p V - vRT p A V = vR AT (because p is constant)
So,	Ljks/.JJAT,
Hence,	A T« (p0 A S + mg) 0-9 К
R
2.11 (a) p = p0 - a V2 = p0 - a
(as, V = RT/p for one mole of gas)
ThUS’	T= RV^py/Po~P ° ^PoP2-P*
For Tmax>
(1)
d(PoP2-/)
—'	' must be zero
dp
190
2.12
2.13
which yields,
2 p-3P0
T 1	2	2 г/Рок/рГ
’	r““" я7ГзРо	" 3 Д
(b)p-P0e-₽y -poe-₽^ so	ёИ.. ln andT- £- In —
P P	$R P
dT For Tmax the condition is — - 0 , which yields
Hence using this value of p in Eq. (1), we get
T -““ e$R
_	p2 7*2
Г- T0 + a V2- TQ + a—i— P (as, V« RT/p for one mole of gas)
So,	p-Va PT(T-P0)1/2
For	р^а, & - 0, which gives
T - 2T0
From (1) and (2), we get,
Pmin- ^ягТоСгТо-То)-172- 2RV^
(2)
(i)
(2)
Consider a thin layer at a height h and thickness dh. Let p and dp +p be the pressure on the two sides of the layer. The mass of the layer is Sdhp. Equating vertical downward force to the upward force acting on the layer.
Sdh p g + (p + dp) 5 - pS
*’ (1)
But, -fjAT, we have ф* M	M
or, ^^dT» pgdh M
So>	-34K/km
dh R
That means, temperature of air drops by 34°CLat a height of 1 km above bottom.
191
2.14 We have, = - pg (See 2.13)
But, from
p  Cpn (where C is, a const) - Cn pn ‘1
(1)
(2)
We have from gas low
p
p* p — T, so using (2) Az
_ Я	«г*	rp	fi П — 1
Cp “ PM T’ 01 T~ ~RCp
2.15
Thus,
But,
So,
dT dh
We have, dp «
dT M f, f 4 \ n -
dT dT dp dp dh dp dp dh
K	Cnp 1
- p g dh and from gas law p «
M
RTP
(3)
nR
(1)
Thus
p RT
Integrating, we get
or,
p	h
f %--iff'* “•
J p RT J	p0 RT
P.	°
(where pQ is the pressure at the surface of the Earth.)
d = d e-Mgh/RT P Poe > •
[Under standard condition, pQ a 1 atm, T = 273 К
Pressure at a height of 5 atm = lx e“28 x 9 81x5000/8314 x 273 s 9.5 atm.
Pressure in a mine at a depth of 5 km = lx e~28 x 9'81x(-500°)/8314x273 e 2 atm.]
2.16
We have dp  - pg dh but from gas law p «
M
Thus
dp »
M
RT nt const, temperature
So,
Integrating witbin limits
^-dh p RT
p	*
f^dh p J RT 0
₽0
192
or,
So, p- Poe-M^randA- -^ln-E-Mg Po
(a)	Given T- 273eK, у - e
Thus	h - -77-lne	- 8 km.
Mg
(b)	T - 273* К and
- 0.01 or -6- - 0.99 Po	Po
RT о
Thus	h - -77- In-*— » 0.09 km on substitution
Mg PO
2.17 From the Barometric formula, we have
p-poe-^
and from gas law
P“ RT
So, at constant temperature from these two Eqs.
p.*,’»*". Poe-"«“r	(1)
Jtxl
Eq. (1) shows that density varies with height in the same manner as pressure. Let us consider the mass element of the gas contained in the colhmn.
dm- p(5dA)- ^.e-Mgh/ltTSdh KI
Hence the sought mass, h 0
2.18 As the gravitational field is constant the centre of gravity and the centre of mass are same. The location of C.M.
h =
hdm
hpdh
0 co
But from Barometric formula and gas law p » p0 e~M%h/RT
193
So,
f h(e‘Mgh/RT\dh
*o= RT_ a	Mg
/ ^e-^RT^dh
2.19 (a) We know that the variation of pressure with height of a fluid is given by : Ф= -Pgdh
But from gas law
p = mrt or> P = 1f
From these two Eqs.
dp* ~^r~dh
Ixl
or,
dp P
-Mgdh
RT^l-ah)
h
Integrating,
pq
g I dh
?- I -7\-----7T, we get
0 J (1 - ah)
0
ln£ - 1п(1-аЛ)Мг/вКГ»
P$
Hence,	p « p0 (1 - ah)Mg/aR\ Obvionsly h < ~
(b) Proceed up to Eq. (1) of part (a), and then put T « TQ (1 + a h) and proceed further in the same fashion to get
P~ (W*
2.20 Let us consider the mass element of the gas (thin layer) in the cylinder at a distance r from its open end as shown in the figure.
Using Newton’s second law for the element Fn - mWn‘
(p + dp) S -pS = (p S dr) co2 r
or, dp- p co2 r dr =	; co2 r dr
KT
194
*	г
_	dp Мы2 , Г dp Мш2 С .
So,	г dr or, I -х-« I г dr,
p RT	J p RT J ’
P'	0
Thus, lnJ”- 0Г’ P~ PoeMU> "^2RT
?.21 For an ideal gas law
So,	p - 0-082 x 300 x atms » 279-5 atmosphere
For Vander Waal gas Eq.
( Ja\
Ip + (V- v b) « v R T, where V « vVM
yRT ay2 ~ mRT/M am2
Or’	P“ V-vb'v7” mb ~v2M2
M
2.22
(a) P
pRT ap2
M-pb~ m2
79-2 atm
V»-b'v’ <ltn)
RT
Vu
(The pressure is less for a Vander Waal gas than for an ideal gas)
p 7*	1 M_____
VM(VM-b)
or,
g(i + n)
V2
+ -1±IL vm VM~b
or,
a(l+Tj) (Vw-b)
T«»	.—77----Гт-, (here Vu is the molar volume.)
1-35 x 1 1 x (1 - 0 039)	„
0-082 x (0-139)	” Z5K
(b) The corresponding pressure is
л RT a a (1 + ti) a Ум(П^ + Ь)’^
а (Ум + ЧУм-ЧУн-Ь') g (VM-b)
Ж V2 (nVu + b) “ V2(VM + b)
135 0-961	,
’ “1“ <M39“9'3 “
195
гв л-кт,^-^,Р2.птг^--^
So, or. Also,
or,
P2-P1- v_b
R(T2-T,)	R(T,-T)
V-b----—   or, fr-V-—-2--------11
P2-P1	P2-P1
P2-P1 a
P1~ 21T2-Tj~ у2’
a _ Pi (P2 ~Pi) P1P2 ~Pi P2
V^’ T2-Tt Pi" T2-T\
у 2 Р1Р2~Р1Р2 P2-P1
Using Tx - 300 K, » 90 atms, T2  350 K, p2 » 110 atm, V * 0-250 litre
a - 1-87 atm. litre2/mole2, b - 0-045 litre/mole
2.24
RT а „(др\ RTV 2a v-ь у1 |av|r (v-ь)2 v2
or,
RTV3-laty-bfV' V2{V-b)
V2(V-b)2	’ [ RTV3 - 2a (V-fr)21
2.25
For an ideal gas k0
V RT
кг (У^ЬУ
Now к - -
RTV
1_2a(V-b)2
RTV3
— 1
Л fr?
= K° I1" V
2
2a ( b\
1	1 - ~I
RTV\ У)
f< 2a ]	, ..	.	,
k0 J1 - — + I, to leading order m a, b
Now
кж0
2a 2b - a
RTV9 V °r T<bR
If a, b do not vary much with temperature, then the effect at high temperature is clearly determined by b and its effect is repulsive so compressibility is less.
196
2.2 THE FIRST LAW OF THERMODYNAMICS. HEAT CAPACITY
2.26
Internal energy of air, treating as an ideal gas
U = — CVT = — T = M v My-l Y-l
R	c,
q . ---- since C - Cv » R and тг- e Y
v у -1	p v Cy
Using
(1)
Thus at constant pressure U » constant, because the volume of the room is a constant.
Puting the value of p «patm and V in Eq. (1), we get U « 10 MJ.
2.27
From eneigy conservation
U, + |(vA/)v2 = Uf
or,
(1)
But from U = v , At/ = Y-l
Hence from Eqs. (1) and (2).
vR
-—- AT (from the previous problem)
(2)
AT
_ W(y-i)
2R
&U = ^vMv2
2.28 On opening the valve, the air will flow from the vessel at heigher pressure to the vessel at lower pressure till both vessels have the same air pressure. If this air pressure is p, the total volume of the air in the two vessels will be (V^ + V2). Also if and v2 be the number of moles of air initially in the two vessels, we have
Pl V1 “ V1RT1 and P2 V2 = V2RT2	C1)
After the air is mixed up, the total number of moles are (vx + v2) and the mixture is at •temperature T.
Hence	p (+ V2) = (vx + v2) RT	(2)
Let us look at the two portions of air as one single system. Since this system is contained in a thermally insulated vessel, no heat exchange is involved in the process. That is, total heat transfer for the combined system 2=0
Moreover, this combined system does not perform mechanical work either. The walls of the containers are rigid and there are no pistons etc to be pushed, looking at the total system, we know A = 0.
Hence, internal energy of the combined system docs not change in the process. Initially energy of the combined system is equal to the sum of internal energies of the two portions of air :
Ut~ u\ + u2 =
V1 v2^ ^2
Y-l + Y-l
(3)
197
Final internal energy of (nt + n^) moles of air at temperature T is given by
(v1 + v2)7?T
Uf= -------;---
т	y-l
(4)
Therefore, Ui« Uj implies :
T_ V17'1 + V2T2_	P1V1+P2V2 _тт Р1^+Л^2
v1 + v2 =	(p2V2/T2)= 1 2PlVtT^p2V2T2
From (2), therefore, final pressure is given by :
V1*V2 пт . R ... т	PlVl+P2V2
P V1 + V2RT У1 + У2 1Г1	V1 + V2
This process in an example of free adiabatic expansion of ideal gas.
2.29	By the first law of thermodynamics, 2- AU + A
Here A » 0, as the volume remains constant, v R
So,	2=ДС7=-^-ДТ
Y-l
From gas law,	p0 V = v R To
So, ДС7 =	---ж-0-25 kJ
To (Y ” !)
Hence amount of heat lost = - ДС7 = 0.25 kJ
2.30	By the first law of thermodynamics Q = AU + A p AV A
But	AU » —— « ——- (as p is constant)
e= -^- + A=	-~^--x2 = 7 J
y-l y-l 1*4-1
2.31
Under isobaric process A = pAV « R AT (as v = 1) = 0-6 kJ From the first law of thermodynamics
AU = g-A = Q~RAT = IkJ
R AT
Again increment in internal energy AU = --, for v = 1
Thus
2.32	Let v « 2 moles of the gas. In the first phase, under isochoric process, Ax = 0, therefore from gas law if pressure is reduced n times so that temperature i.e. new temperature becomes Tq/п.
Now from first law of thermodynamics
Qis AUr-
vRAT
Y-l
198
ж	уДГ0(1-п)
Y - 1 п °) п (y - 1) During the second phase (under isobaric process), A2« рду. v R AT
Thus from first law of thermodynamics :
e2- ДУ2 +Л2- ^y + vtfAT о (т
_ уДг°~ "Г_ v/?T0(»-l)Y Y-l ’	«(Y-l)
Hence the total amount of heat absorbed л л л уДГ0(1-и) уЯТ0(”-РГ Q Qi + Q2	п (у ~ 1) +	п (y -1)
vRT0(n-l)y
---(- 1 + y) - vRT0 л (y-l)
2.33	Total no. of moles of the mixture v « vx + v2
At a certain temperature, U « Ux + U2 or v Cv« Vj Cv + v2 Cv*
Thus
Similarly
Thus
I R R \ у.---r + v2---?!
V1^V+V2^V	\
-----L_ L------------
V1Y1<Y2~1) + V2Y2<Y1-1)
V1 (Y2 - 1) + V2 (Y1 - 1)
l.'M	previous problem
R R
V.---- + v2-----г
1 Yi -1	2 Yi -1
c	_2J--------. 15-2J/mole.K
V	Vl+V2
199
У1Я y2R vi----7 + v2-----7
1 Yi - 1 Yi_ 1 and	C, - —12----------------- 23-85 J/mole. К
P	vl + v2
kt i	Z1Z4 Total mass	20 + 7
Now molar mass of the mixture (M) - ——:---:-------  — -   —
Total number of moles 1 1 — + — 2 4
C	C
Hence cv= 0-42 J/g-К and cp~ ~£ = 0-66J/g-K
2.35 Let S be the area of the piston and F be the force exerted by the external agent Then, F +pS- p0S (Fig.) at an arbitrary instant of time. Herep is the pressure at the instant the volume is V. (Initially the pressure inside is p^
A (Work done by the agent)» J* Fdx

0
"''о

= Po(n-l)Vo-J pdV»p0(n-l)V0 J vRT-~ vo	vo
« (т) - 1)pQ Vq - nRT In T) * (rj -1) vRT - vRT In rj
= vRT (r] - 1 - In t|) = RT (r) - 1 - In t]) (For v » 1 mole)
2.36 Let the agent move the piston to the right by r. In equilibirium position, PlS+Fagml-p2S, 0I> F«gauB (P2-P^S
Work done by the agent in an infmitesmal change dx is (P2-Pl)S<fa“ (Р2-Р1^
By applying pV - constant, for the two parts,
Pi (Vo + Sx) - p0 vo and P2 (Vo - Sx) - p0 Vo
p0V02Sx 2p0V0V
So,	P2 -pz - V2_S2J -	(wherC Sx Ж
When the volume of the left end is T] times the volume of the right end (Vo+ V)» T) (Vo - V), or, V= Vo
200
v	v
A~f<P2 -Л)	-P0 vo Г b (Vo2 - v2)
о	о 0	L
- -PoVo[ln(Vo2-V2)-lnV2]
- -Po vo ln
V2 f-D-------1_ \ y2
v0 1 2 Vo hr] + 1 I
-In Vo2
- -p0 Vo (in 4т] , К p0 VoIn
° °( (n + 1) J 4n
2.37 In the isothermal process, heat transfer to the gas is given by
V2	(	V2 Pl\
21-vPToln — - vRTQ]nr] Fort]-—« — F1	V1 P2)
In the isochoric process, A « 0 Thus heat transfer to the gas is given by 2,- ДС7 - vCvAT- -^-AT (for Cv-Y-l I Y-l)
к, .	Pa	Pi г (f Pi |
But T“T’ 0Г’ ГжГо —-nT0 Horn- —
Pi 1	P2 ( Pa J
vR or, AT- т]Го-То= (n-l)To. so, Q2- — 	- 1) To
Thus, net heat transfer to the gas vP
2 = уЯТв1пЛ + ^-(п-1)То
2	, n -1 Q , n -1
or,	= In n + -1——, or, —- In n = -1——
vRT0 1 y-l vRTq 1 y-l or, y “ 1 +	~ --• 1 + 7-------~~з~—\-----“ 1‘4
-fer-lnn	—-gQ-M,03,   _ln6
vPT0	13 x 8-314 x 273 I
2.38 (a) From ideal gas law p « j Г = kT ^where к » —j
For isochoric process, obviously к = constant, thusp « kT, represents a straight line passing through the origin and its slope becomes k.
For isobaric processp « constant, thus onp - T curve, it is a horizontal straight line parallel to T - axis, if T is along horizontal (or x - axis)
For isothermal process, T« constant, thus on p - T curve, it represents a vertical straight line if T is taken along horizontal (or x - axis)
For adiabatic process T7p1-7 = constant
After diffrentiating, we get (1 - y)p~v dp • Tv + yp1 ~7 •	1 • dT = 0
201
/ у	(Y \p
dT~ [i-у)(p~Y I F J V 1/T
The approximate plots of isochoric, isobaric, isothermal, and adiabatic processess are drawn in the answersheet.
(b) As p is not considered as variable, we have from ideal gas law
V= — T~ XTLhereЦ. —1 P к P)
On V - T co-ordinate system let us, take T along x - axis.
For isochoric process V « constant, thus k!  constant and V• k'T obviously represents a straight line pasing through the origin of the co-ordinate system and kf is its slope.
For isothermal process T • constant. Thus on the stated co- ordinate system it represents a straight line parallel to the V - axis.
For adiabatic process TV1 ~1 • constant
After differentiating, we get (y - 1) VY ~ 2 dV • T + И “1 dT • 0
dV _ / 1 \ V dT*	T
The approximate plots of isochoric, isobaric, isothermal and adiabatic processess are drawn in the answer sheet
2.39 According to T-p relation in adiabatic process, 7* - kp^~^ (where к - constant)
and	» [—]	So, — • if-1 (for rj - ~)
W W П I, /’J
Hence T - Ta • n = 290 x 10( 14'1)Z1'4 - 0-56 kK Y
(b) Using the solution of part (a), sought work done
A « A- « -—~ (t)( y “1)/Y -	« 5-61 kJ (on substitution)
2.40 Let (p0, Vq, Tq) be the initial state of the gas.
We know	(work done by the gas)
But from the equation TVY-1 = constant, we get AT= T0^Y-1
"adia
Thus
Y-l
On the other hand, we know Abo ® vRT0 In = - vR TQ In T) (work done by the gas)
Aiso (Y - 1) In t] 0-4 x In 5
Thus
202
2.41 Since here the piston is conducting and it is moved slowly the temperature on the two sides increases and maintained at the same value.
Elementary work done by the agent = Work done in compression - Work done in expansion i.e. <14- p2dV-pxdV^ (p2”jPi)^
where pY and p2 are pressures at any instant of the gas on expansion and compression side respectively.
From the gas law pr (Vo + Sx) » vRT and p2 (Vo - Sx) « vRT, for each section
(x is the displacement of the piston towards section 2)
? Vr	9V
So, p2 -p. - V/?T - x--	9 - vRT~—z (as Sx - V)
2	vg-S2j? Vq-V2
2V <Z4 - vRT . a dV vj-v2
Also, from the first law of thermodynamics dA = -dU = -2v^-^dT (as dQ~ 0)
So
p
So, work done on the gas = - dA » 2v-- dT
Y-l
p
Thus 2v——-dT = Y-l
2V-dV vRT—z---x,
v2-v2
VdV
Vo2 - V2
When the left end is t) times the volume of the right end.
or,
dT 1 T-Y-l
(Vo + V) = n(Vo-v)

т
V
On integrating
I dT , I	VdV
J	^5
T„	0
or
Hence
Fagent У	^-Pes
ln^= (Y-l)[-|ln(V2-V2)
7o I z	Jo
= - ^ [ln (vo2 - v2) - In v2 - V2) - In V2 ]
2
2	4т]
203
2.42 From energy conservation as in the derivation of Bemoullis theorem it reads
2.
P
+ gz + и + Qd » constant
(1)
In the Eq. (1) и is the internal eneigy per unit mass and in this case is the thermal eneigy per unit mass of the gas. As the gas vessel is thermally insulated Qd « 0, also in our case.
CVT RT	p RT
Just inside the vessel и « — « -777-77 also » — • Inside the vessel v * 0 also. Just
M	M(y-l)	p	M
outside p « 0, and и « 0. Ingeneral gz is not very significant for gases.
Thus applying Eq. (1) just inside and outside the hole, we get
1	2 P — v =	+ и
2	p
RT RT yRT
M +M(y-1) “ M(y- 1)
2 2y AT	4 / 2yRT п 00 1 !
Hence v « -777-----tv or, v « v -777---тт я 3.22 km/s.
M (у- 1)	▼ M (у- 1)
Note : The velocity here is the velocity of hydrodynamic flow of the gas into vaccum. This requires that the diameter of the hole is not too small (D > mean free path I). In the opposite case (D < < /) the flow is called effusion. Then the above result does not apply and kinetic theory methods are needed.
2.43	The differential work done by the gas
dA- pdV-
^as pV» vRT and V- yj
T+AT
So,	A = vRdT = - у RAT
т
From the first law of thermodynamics
v R
Q~ AU + A~ -^-AT-vRAT
Y-l
=	(for v= 1 mole)
Y-l	Y-l
2.44	According to the problem : A a U or dA = aU (where a is proportionality constant) avRdT	...
or,	P<W- Y_1	(1)
From ideal gas law, pV« vRT, on differentiating pdV+Vdp= vRdT	(2)
204
Thus from (1) and (2) pdV- -^(pdV+Vdp)
or, pdV I fl . - 11 + ~~ V dp “ 0
I у -1	I Y “ 1
or,
pdV (k - 1) + kVdp - 0 (where к -	- another constant)
or,
к - 1 T,,	„
-j- +Vdp- 0
or,
£ 1 pdVn + Vdp - 0 (where —-— « n » ratio)
Dividing both the sides by pV dV dp n n — + -^- 0
V P
On integrating n In V+ In p - In C (where C is constant)
In (pVH) - In C or, pVn - C (const)
or,
2.45
In the polytropic process work done by the gas vMTf-TJ A « -----------------------------------—1—
n- 1
(where Tt- and are initial and final temperature of the gas like in adiabatic process)
and	A17 =
By the first law of thermodynamics Q - AU + A
vR	vR
- (Tf-T,)vR
_1_____L_l.	дг
Y - 1 и -1 (и -1) (y - 1)
According to definition of molar heat capacity when number of moles v = 1 and AT = 1 then Q = Molar heat capacity.
Here,	Cn - ; -	-- - <0 for 1 < n < у
я (и-1)(у-1)
2-46 Let the process be polytropic according to the law pVn = constant
Thus,	PfVf^PiV" or, |—|-0
\Pf)
So,	a" = 6 or Infi = nIna or n = 7^-
r	In a
In the polytropic process molar heat capacity is given by
205
So,
С,-
я R(n-y) R____________R_
(м-1)(у-1)“ у-I "n-1
R R In a , In P
- ----— ------- where n« 7-
Y-l In P - In a	In a
8-314	8-314 In 4
1-66 - 1 In 8 - In 4
-42J/mol.K
2.47 (a) Increment of internal energy for AT, becomes
,TT vRAT RAT T, ,	14
AU = ---— = ---- = - 324 J (as v = 1 mole)
Y-l Y-l
From first law of thermodynamics _	. rr . RAT RAT n,,.T
2- AU+A - -—---—7- 0-11 kJ
y-l n- 1 vt
dV
v 1
(where pVn - к - p, V- - pf Vj)
к
1 - n
1 -n vRlTf-T,) 1 -n
vR^T n-1
2.48 LaW of the process is p
so the process is polytropic of index n
As p
(a)
(b)
PfVf-PiVj 1 - n
« - -« 0-43 kJ (as v - 1 mole) n — 1
 a V or pV~1« a
-1
aV so, Pi - a Vo and py = a rj Vo
Increment of the internal energy is given by
At/- [Tz - T; ] -	—
y-l 1 J ,J y-l
Work done by the gas is given by
A_ Ppi ~PfVf _	Vp
(c)
n-1	-1-1
аУ02(1-т]2) -2 Molar heat capacity is given by
Л(л-у) *(-l-Y)	* Y + l
(n-l)(Y-l)“ (-1-1)(y-1)" 2y-l
|<xV2(n2-l)
206
2.49 (a) AC7- -^ДТ and Q- vC„AT
x ' Y -1	л
where Cn is the molar heat capacity in the process. It is given that Q - - AU
So,	С АТ» —ДТ, or C-"	y-l	" y-l
(b) or,	By the first law of thermodynamics, dQ • dU + dA, 2dQ~ dA (as dQ^ -dU) 2Rv 2vCndT= pdV, or,	pdV = 0
So,	2RV	vRT „r .	2 dT dV n . dT+	dV= 0, or, z	+ rr = 0 Y-l	V	’	’ (y — 1) T V
or,	T +	— у « 0, or, 7V^Y 1^/2 - constant
(c) We know C - 7—
V	" (и - 1) (у - 1)
2^
But from part (a), we have Cn = - -—-
Thus	- -----=	. which yields
Y-l (n - 1) (y - 1)
From part (b); we know	e constant
To (V\{^1V2
So, -£ • кт-i «	(where T is the final temperature)
Work done by the gas for one mole is given by
(To-T) 2RT0[l-^-^] A = R------— « ---------------
n-1	Y“ 1
2.50	Given p » a Ta (for one mole of gas)
So,	pT~a = a or,	e л»
or,	pr~av~a^ aR~a or,	constant
Here polytropic exponent n = ---y
(a)	In the polytropic process for one mole of gas :
A _	_ ДАГ  = ядт(1 - a)
1 - n Л a \
\ ”a”1/
(b)	Molar heat capacity is given by
c- —------------ —----------------- -^-r + R(l-a)
y-l n -1 y-l a _. y-l
a-1
207
2.51	Given £7- aVa
or,	vCvT-aV“, or, vCv^-aV“
v	v vR
__a R 1	.	Tza-l -1 ^V
or,	aV-----77» 1, or» Va -p » —
Cv pV	Ra
or	pV1-a- constant- a(y-l) as Cv- - -
Cv	у -1
So polytropric index n = 1 - a.
(a)	Work done by the gas is given by
л -vRAT J лгг vRAT A = --;— and AU» -r-
и- 1	у- 1
Непсе	(„
n -1	a
By the first law of thermodynamics, Q « AU+A = A£7+At/h+IrJ. a	a
(b)	Molar heat capacity is given by R R R R C"y-i~n-l“y-1 1-a-l
R R z 4	4
= ---- + — (as n « 1 - a)
y-1 a
2.52	(a) By the first law .of thermodynamics
Jg- dU + dA- vCvdT+pdV
Molar specific heat according to definition
dQ CvdT+pdV C“ vdT~ vdT
vCvdT+ у dV RTdV vdT “ Су+ V dT
We have
So,
After differentiating, we get dT dV DT
T- ToeaV . aToeaV-dV
Hence
C,
RT 1
V 'aT/v
nqr _ a V	_
Q +____2----ш (J +J^—
v aVToeav v aV
(b) Process is p - p0 e
RT aV
P- ~y-Poe
208
or, T-^eaV-V
О Г - Г	— Г -Ln Z»aV ____—____ Г -L —
So, C-Cv + y dT-Cv+Poe poeav(1+ay)= Cv+i
2.53 Using 2.52
(a) C= Cv+^^ = Cv + Scif (for onc mole of Sas)
,.z	a RT a	и
Wehavep-p0 + - or, —= p0+y> or> ЛГвРоу+а
Therefore	RdT • podV, So, —
ai pQ
Hence	C- Cv+L + ^V —- -^-+fl+-^U
V V) Po T-1 ( P°V)
(n R \ aR yR aR
Y-1J P0V Y-l Pov
(b) Work done is given by
A-J L + ^-Po(^-^) + «b^
(p'2 ^2 Pl
At/= CV(T2- Г) = Cv(for one mole) l К ЛА 1
^r^-P^
2.54
YZT[(Po + aV2)V2-
г °+ vi r1 “ ~
By the first law of thermodynamics Q - AU +A Vi Po^-VA
YPo(^2-^	. V2
“ -----i---+ «ln v-
Y-l V\
(a) Heat capacity is given by
c-c +^^-v V dT

Y-l
(see solution of 2.52)
We have
T Tq
T-T0 + aV or, V-±- — u	a a
dV 1
After differentiating, we get, — » —
209
Непсе
r r 1
C" Cv+ V 'a“ y-l
*(T0 + aV) 1
V a
R Y-l
.xREo r y-l a V ” v aV
RT0 aV
(b) Given T- T0 + aV
pV
As T «	for one mole of gas
R
P~ ^(To + aV)- J’ a«
2
Now
dV (for one mole)
Vi
&U- Cyi^-Ty)
- Cv\T0 + aV2-T0aVy\- o.Cv(V2-Vy)
By the first law of thermodynamics Q  AC7+A
ш ^0Г2-^1)+ЛГо1п^+аЛ(Г2-У1)
Г 1 1	v2
<*R(V2-VJ I+-7- +RT0\n^
1
Y-l
V2 - aCp(V2-V1+RT0\n^
V2
- aC/^-V^+AToln^
2.55 „	... v „ r. RTdV
Heat capacity is given by C - Cv+ ~y"^
(a) Given C - Cv+aT
_	_	_	~ RT dV a , dV
So, Су + aT ж Су + у дт or> dTж у
Integrating both sides, we get -^T « In V + In Co « In VC0, Co is R
V-Co- eaT/R or У-еоГ/л- constant co
constant
Or,
(b) C= Cv+₽V
210
and
, RTdV RTdV „ o„ v+ V dT S0’ Cv V dT° Cv+$V
RTdV OT7 dV bdT TZ_2 dT V dT к у2 R T 9	T
R V"1
Integrating both sides, we get —	- In T + In Co • In T*C0
T-Co- e-^v
or,
D
So, hT-C.--^
or,
T e _R/^V - -i- - constant Co
(с) C- Cv + ap and C- Cv + ^~
„	„ RTdV	RTdV
Cv + ap~ Cv+ — — so, ap~——
So,
or,
RT RTdV, RT r , r . a — - — — (as p = — for one mole of gas)
or,
— - a or, av» adT or, dT« — dT	a
у
So,T « — + constant or V - gT « constant a
2.56 (a) By the first law of thermodynamics A « Q - A(7
CdT -CvdT= (C - Cv) dT (for one mole)
or,
Given
C--T
So.
A«
ro
n Tn dT- a1n-!/-Cv(n To-To) 70
RT
- а1пт]-СгГ0(т|-1) == alnrj +
(b) c
Given
dQ RTdV-dT~ V dT v
„ а _ RT dV а
c= ?’ so ^+V^"7
or,
or,
7?__1_ dV a
у-1АГ+ VШ
dV a 1 dT
V~ rt> 'y-1 T
or,
(Y ' v RT2 r

Integrating both sides, we get
211
or,	(y-l)lnV= -Ct(I-1^-lnT + lnA'
KT
or,
InV7-1 J-К RT
In V7
-1 £V RK
- a (y -1)
pv
0Г,
or, 2.57 The work done is
P^ , _-a(Y-IVpV
RK a(y-l)/,V pVy - RK » constant
2.58 (a) The increment in the internal energy is
But from second law
On the other hand
RT a
? V-b y2
(b) From the first law
V2-b 2« A + A17= RT In -7—7
2.59 (a) From the first law for an adiabatic
dQ = dU+pdV = 0
From the previous problem
</£/- (ттй d7’+f^ dv" CvdT+\dV
So,	0-CvdT+y^.
212
This equation can be integrated if we assume that Cv and b are constant then
R dV dT л , rr R , ,X7 . 4
In T+—In (V - h) « constant
dT
CvV-b+T~0, or’
or,
(b) We use
T(V-b)R/Cv^ constant
dU- CvdT+4zdV
v y2
Now,
RT dQ- CvdT + ^dV
So along constant p,
Thus
_	_ a RT (&V\
p~ v v-blar \ ip
’ Butp“ V-b~ И
RT V-b
Ce-C
.... л ( RT 2a\(dV\ R
On differentiating, 0 = --------z + tz +17—7
(v-ь)2	v~b
or,
T
RT/V-b
RT 2a" (V-b)2 V3
V-b t 2a (V-b)2 RTV3
and
cB-cv~-----------«
p	2a (V-b)2
RTV*
2.60 From the first law
Q - Uf - Ui + A « 0, as the vessels are themally insulated.
As this is free expansion, A « 0, so, Uf « Ui
av2 U-vCvT~ —
But
So,
or,
la a\ ~«V,v
+	—j v -	+
>T -fl(Y~l)^2v RVr(V1 + V2)
Substitution gives A T - - 3 К
2.61 2 s Uf - Ui + A « Uf-U^ (as A = 0 in free expansion). So at constant temperature.
- av2
Q~ v2 yj" V1-V2
- 0*33 kJ from the given data.
213
2.3 KINETIC THEORY OF GASES. BOLTZMANN’S LAW AND MAXWELL’S DISTRIBUTION
2.62
From the formulap» nkT
P kT
n =
4 x 10'15 x 1-01 x 10s
1’38 x 10” 23 x 300
per m3
 lx 1011 per m3 - 105 per c.c Mean distance between molecules
(10'5c.c.)1/3 - 101/3 x 10‘2 cm - 0-2 mm.
2.63 After dissociation each N2 molecule becomes two N- a toms and so contributes, 2x3 degrees of freedom. Thus the number of moles becomes
andp.^(l + 11)
Here M is the molecular weight in grams of
2.64 Let nr « number density of He atoms, n2 - number density of N2 molecules
Then	p = nY + n2 m2
where - mass of He atom, m2 - mass of N2 molecule also p - (nx + n2) kT From these two equations we get
If i = number of degrees of freedom of the gas then
CV + RT and Cv~ ^RT
2.67
214
м
For monoatomic gases i - 3
(b)
2.68
2.69
VT- 0-75
Vrms
For rigid diatomic molecules i» 5
- 0-68
Vrms V И
For a general noncollinear, nonplanar molecule 3	3
mean energy « ~ k T (translational) + — kT (rotational) + (3 N - 6) kT (vibrational)
= (3N - 3) kT per molecule
3
For linear molecules, mean energy » — kT (translational)
+ kT (rotational) + (3N - 5) kT (vibrational)
» 13 N - || kT per molecule
Translational energy is a fraction	—7T and ——~ in the two cases.
2vv“1'	2У-|
(a)	A diatomic molecule has 3 translational, 2 rotational and one vibrational degrees of freedom. The corresponding energy per mole is
3	1
—AT, (for translational) + 2 x—AT, (for rotational)
7
+ 1 x AT, (for vibrational) » —AT
Thus,
7	CP 9
Cv= —R, and Y» 7
(b)	For linear N- atomic molecules energy per mole = (3Ar-|]flTas before
Cv —	and
(c) For noncoll inear N- atomic molecules 3V- 2
Cv= 3 (N- 1) A as before (268) у s
So,
6У-2 6ЛГ-5
7V-2/3 TV-1
2.70 In the isobaric process, work done is
A « pdv « RdT per mole.
On the other hand heat transferred Q- CpdT
Now Cp = (3N -2)R for non-collinear molecules and Cp =
'	3\
ЗА-- Яfw ^ear mo^ecu^es
215
Thus
1
——— non collinear 37v - 2
1
-----—linear
“-2
For monoatomic gases, cp -
| and
A	2
— SB -
Q	5
2.71 Given specific beats cf, cv (per unit mass)
D
M(c-cv)-R or, Л/- ———
P v'	c -c
p V
Also
J 29
2-72 <a> C» = 294C=T 8GR
< = 5
(b) In the process pT - const
j.2
— = const,
So
T V ~
0
RT	2RT
Thus CdT- CvdT+pdV= CvdT+^dV-CvdT + ^dT
/29 \	12-4	3
or	C-CV+2R-	So CV-^R‘1R
I vS* J J	U*3	£
Hence i « 3 (monoatomic)
2.73	Obviously
1^3	5
ACv= 2V1 + 2Y2
3	5
(Since a monoatomic gas has Cv- — 7? and a diatomic gas has Cv- —R. [The diatomic molecule is rigid so no vibration])
1 „	3	5
RCp= 2Y1 + 2Y2 + Y1+Y2
Hence
3 Y1 + 5 y2
2.74	The internal energy of the molecules are
U » ^-mN <(u^- v^2>« ^mN <u2 - v2>
216
where velocity of the vessel, N = number of molecules, each of mass m. When the 1	2
vessel is stopped, internal energy becomes — mN <u >
1	2
So there is an increase in internal eneigy of &U = -mNv. This will give rise to a rise in temperature of
\mNv2
mN v' iR
there being no flow of heat This change of temperature will lead to an excess pressure
. R&T mNv2
-----iv~
and finally	= 2-2 %
p iR T
where M = molecular weight of N2, i* number of degrees of freedom of N2
2.75	(a) From the equipartition theorem
—	r in-21 т л	ч [ЗкТ - /3 Я T	/
е» —kT - 6 х 10 J; and vrmx« v------- - V ж 0*47km/s
2	rms v m V м
(b) In equilibrium the mean kinetic energy of the droplet will be equal to that of a molecule.
1 л a 2	3 . _	a- 12kT _
26^Pv--2^Z °r V™“3V^77 - 045m/s
,	i iч/з7Гт - i T
rms У M rj rms T| v M	t|2
Now in an adiabatic process
TV7 -1« 7 у27'« constant or V Ti/2 « constant
/1	\^2
V -^T - VT72 h I
or V - V or
V - г)1' V
The gas must be expanded tj* times, i.e 7-6 times.
2.77 Here Cv- ~R(i- 5 here)
2 M
m= mass of the gas, Af= molecular weight If vrms increases T) times, the temperature will have increased retimes. This will require (neglecting expansion of the vessels) a heat flow of amount
|£я(П2-1)Т- 10 kJ.
2 M
217
2.78	The root mean square angular velocity is given by 1 2 1
—Zco » 2x- kT (2 degrees of rotations)
or	co »	= 6-3 x 1012 rad/s
2.79	Under compression, the temperature will rise
TV7"1» constant, TV 2/1 = constant
or,	ПтГЧ)2^ or, r=n + 2/' T0
So mean kinetic energy of rotation per molecule in the compressed state « kT - JtT’oT)2''' - 0-72 x IO"20 J
2.80	No. of collisions » — л <v> » v
(When the gas is expanded v| times, n decreases by a factor T|). Also
7* (r]V)2/‘.. TV24 or T-r\ViT so,
i+1
i.e. collisions decrease by a factor T] * , i • 5 here.
in a polytropic process pVn - constant., where n is called the polytropic index. For this process
pVn » constant or TV""1» constant c/T /	. x <TV n
т + (л-1) —- 0
Then	dQ = CdT= dU+pdV= CvdT+pdV
= ±RdT + ^dV= ^RdT--~RdT=
2 V 2 n-l 12 n-1j
Now	C •= R so jr - —^—7 = 1
2 n -1
1 i .	t-2	i
or’
n-l
__	v' n' <v'>	1 - /Т7" 1 (V\ 2
Now	—«-------= - V7 = “77
v n <v> T) v T T] IVI
1	/-1
1 /IV-2 /IV-2 :±il	1
» — —	» —	» n i-2 times » ——times
nln) In)	2‘52
218
2.82 If a is the polytropic index then pVa « constant, 7Va "1 = constant
XT	v' n'<v'> V^/Tr VT-1/2	,
NOW	— =------= —	— = -------777 = 1
V n <V> V ▼ T у p -1/2
YT	1	1
Hence	--г =* - — or a « - 1
a-1	2
_	_ iR R „
Then	C » — + — « 3R
2.83
IkT m
0-45
km/s,
=
vm ж = • 51 km/s av v л p
and
= 0-55 km/s rmj у p
2.84 (a) The formula is
df (u) « -i-м2 e"u du, where и = —
Vn	vp
Now Prob
l + bn
1 -бт]
-3/2
- 0 0185
219
2.85
Vrm.-Vp”	~ AV-
/ А ч2
т- 7(<vrw) /к ' 384К
(b) Clearly v is the most probable speed at this temperature. So
2.86
(a) We have,
T- - 342К 2k
2_ 2ArT_ ^1~у2 °Г Vp“ m “ (Inv^/v^)
•* “	2 “
V, 2fcIn-~-v2
(b) F(v)= -4=’~e~v/*x — f — comes from F(y)dv = d f (h), du «
V7t Vp	vp Vp	vp
2	2 2	OlrT	ЭкТ
Thus -j-e"v/vfl= v2/v e~v^\ v2 = ------v2 « --------q now
v3	‘ Рг	A m ’ m 1
A
2.88
v2. 3kT--He ' H
mH -mH
Putting the values we get v - 1-60 km/s
220
2.89
dN(y)-
N 4 v2 dv _VW
Т7-~з“е	'
Vn vp
For a given range v to v + dv (i.e. given v and dv ) this is maximum when
or,
6 dN(v) _ 0 ® vp N v^ dv
3vJ4 + ^le-v4
2	з	2	Зкт	_	1 mv2
---------Thus	Т»	~—r~ 2	p	m---3 k
2.90 £ v = 2 л v± dvL dvx
3/2
Thus	d n (y) =s N
dv2izv, dv
2.91 <vx > = 0 by symmetry
I vj > =
2 - mv
x
о
e 2kT dvx =
2	Л	2
~mvx	Г -mv
vx e 2кт dvx/ I e 2kT dv3
0
о	0
2.92
<
2.93 Here vdA = No. of molecules hitting an area dA of the wall per second
dN(yx)vxdA
о
221
or,
2 mv
e vx dvx
/•	/	v 1/2
I ( m \
I n “—777
J 12 лкТ I
o' 7
dA
where <v> «
2.94
/	\V2	2
be the number of molecules per unit volume with x component of velocity in the range v to vv + dvv
Then
p-l 2 mvx • vx dn (vx) о
1 2kT f 2 -u1 2 J « 2m«-T=--- I и e du
Vx ™ J о
222
2.96
I \3/2	2
dN(y)~ NT| e~mv/2*T 4 л v2 . dV(e) « dt I Zftxj J	de
or,
X /	.3/2
bA-™—\	-™/2RT 4jtv2dv
de (2^TI e 4JlV de
Now,
12	dv	1
e « -• mv so — « —
2	de mv
or,
3/2	j 
de (2л:£т1	V m m
^N^-(kT)-3/2e-t/Krzi/2 УЛ
i.e.
^(Е)*#тз(Иу3/2е'!/и’ e1Z2 de vn
The most probable kinetic energy is given from
d dN (e) de de *
or,
1 -1/2 - е/ЛГ
2е e
е^,«дг
kT
0 or e « у kT - epr £
The corresponding velocity is v =
* v~
pr
2.91 The mean kinetic energy is 00	00
<«>-Л- tT^-0	0
Thus
|(l + 4n)*T
^(kT)-3/2e-^ e^de Vn
- 2 е_3/2/|\3/22И_ 3^6" е3/2бт) v% \2j	¥ Л
If 6 T] = 1% this gives 0-9 %
00
2.98 M 2 (ja).^ f ^e-^d£ ™ УЛ	J
eo
- ^(kT)'3/2 J е-^кт de(e0»kT) eo
223
2.99
- Л (кТ)-3/2^кТе-^-Ул	T nkl
(In evaluating the integral, we have taken out Ve as Ve^ since the integral is dominated by the lower limit.)
(a) F(v)= Av3e’m/ar
For the most probable value of the velocity
^-0 or 3Av2e-"‘'I/2*T-Av3^e-"vI/2*r- 0
dv	2kT
So,
v,
3kT m
This should be compared with the value v
r2kT ----- for the Maxwellian distribution.
m
1	2
(b) In terms of eneigy, e « — mv
2.100
2.101
F(e)= Av3 e-mvl/2llT—
dz
\3Z2	Л
('w;	V2me
From this the probable eneigy comes out as follows : F (e) « 0 implies
2A ( -t/кт £ -в/иЛ n
—2 e	1=0, or, e = kT
m\ kT j	*
= A —ze m
The number of molecules reaching a unit area of wall at angle between 9 and 9 + J9 to its normal per unit time is
Jv«
v- 0
/*	\3/2	2
I n	I e“wv/2irv3 JvsinOcosO J9x2ji
/	I 2 л ici j
о	x	'
/2Jt7A	i	!2kT \
«л ----- I e“xxdx sin 9 cos 9 J9= л -------I sin 9 cos 9 J 9
Im л I	J	[mn
\	/	о	x z
Similarly the number of molecules reaching the wall (per unit area of the wall with velocities in the interval v to v + dv per unit time is о- */2
J/l (v) -— v cos 0 4л
о- о
224
0- х/2
/•	/	х	3/2
| ( т \ J " 2лкТ
0- 0	'	'
е ’ mv /'2кТ v3 dv sinO cos0 dO x 2л
/	\3/2	2
=	e’mv/2*rv3Jv
2 лкТ]
2.102 If the force exerted is F then the law of variation of concentration with height reads
«(Z)-noe-^ So,	or F- - 9 x IO’20 N
tt i7 л a . R Finn	6ЛТ1ПТ1
2.103 Here F- — <r Apg- 1 or N~ —5---------------*-
6 re Nah a ncfgbph
In the problem, = 1'39 here
По
T • 290K, T) = 2, Л = 4 x 10 ~ 5 m, d » 4 x 10 “ 7 m, g = 9-8 m/s2, A p = 0-2 x 103 kg/m3 and R = 8-31 J/k u	..	6 x 8-31 x 290	x hi 2	n26	£	. „23	, -1
Hence,	N„ •	---——A1	—т x Ю =	6-36 x	10	mole
° л x 64 x 9-8 200 x 4
2.104
concetration of H2
concentration ofN2
e-MHigh/KT e-Mlli-gh/RT
г\ое{м«г-мнг} gh/RT
So more N-, at the bottom, i-« 1.39 here No
2.Ю5 П1(А)_ П1е-т^ь, ^(Л)- п2е~т^/кг
They are equal at a height h where — « тУкТ n2
or Л=------------------
g mi - m2
2.106
At a temperature T the concentration n (e ) varies with height according to n ) = nQe^mgz/kT
This means that the cylinder contains I n (e )
0
noe~mte/kT dt = mg 0
particles per unit area of the base. Clearly this cannot change. Thus и0 kT • pQ » pressure at the bottom of the cylinder must not change with change of temperature.
225
00	00
f mgze~mgz/kt dz	f xe~xdx
2107 TT 0	,To	У.^ Г (2) LT
<U> - —------------------- kT —-------- kT “ kT
J e^mgz/kTdz	J e~xdx
о	0
When there are many kinds of molecules, this formula holds for each kind and the average eneigy
<!j>- ^J‘kT - kT
where a fractional concentration of each kind at the ground level.
2.108	The constant acceleration is equivalent to a pseudo force wherein a concentration gradient is set up. Then
1_n
or
ATlnlbll) =	= 70g
MAl MAl 8
2.109	In a centrifuge rotating with angular velocity co about an axis, there is a centrifugal acceleration co2 r where r is the radial distance from the axis. In a fluid if there are suspended colloidal particles they experience an additional force. If m is the mass of each particle then its volume is “ and the excess force on this particle is
— (p - p0) co2 r outward corresponding to a potential eneigy - y^(p - Po)w2 p
This gives rise to a concentration variation
n(r)- noexp| + j^;(p-p0)(O2r2j
Thus
where
Thus
П	( M z \ 2 / 2	2\ \
('г-'.))
M = Na m is the molecular weight
M. 2£ЯТ1пт]
(p - Po) °>2	- 'l)
The potential eneigy associated with each molecule is : - m to2 r2
2.110
and there is a concentration variation
Thus
Z4	fmco2^	/Af(D2r2\
» 0 = «0 exP 2ЛТ = "° eXp 2/?T
(Mw2l2\
’’-“Р -глТ »	Vj72'"i
226
Using M = 12 + 32= 44gm, / = 100cm, 7?= 8-31 x 107^, T- 300, JV
we get to = 280 radians per second.
2.111
kT a
(b)
Here и (r) = n0 exp
The number of molecules located at the distance between r and r + dr is 2	( ar2\ 9
4 я r drn(r)» 4 л и0 exp I - — I r dr
rpr is given by —г и(г)« 0 or, 2r-^r« 0 or r; The fraction of molecules lying between r and r + dr is 4лr2drи0exp (-ar2//:!)
J* 4 я r2 dr n0 exp (- ar2/kT) о
(c)
3/2
Thus
(d)
So
4 л r2 dr exp
3/2
C dx . .
J
0 ,^3/2
,KT / \3/2	/ 2\
d/V ( a \ A 2 j I ~ ar ] — « —— 4л r drexp—— N \лкТ}	\ kT )
dV » ЛИ—7=]	4 л/dr exp гу-Г-1,
\nkTj	J
, X1/2	/	2\
IX xr I a 1	(-ar I
“p rirj
When T decreases rj times и(0) - nQ will increase rj3/2 times.
2	/Z7
Write U - ar or r« v > so dr Y a
о
2.112
dU
л U dU (IT
so dN = nn 4 я--7= exp —
° a 2<aU F №
» 2яи0а“3/2 UV2 exp dU
The most probable value of U is given by
d /dV\ n ( 1 UV2\ l-U\ TT l,r
du(duj“ °" |2Vu” kT JeXP^Tj °Г’ UP'~ 2kT
From 2.111 (b), the potential energy at the most probable distance is kT.
227
2.4 THE SECOND LAW OF THERMODYNAMICS. ENTROPY
2.113
The efficiency is given by
Ti-T2 n- T1 ,
Now in the two cases the efficiencies are Ti + AT-Zj
Пл = T, + AT ’	71 increased
Tj-^+AT r|z« -----=------, T2 decreased
Thus
2.114
For H2, у - I
Pi Fj = P2 F2, P3 Fj = p4 V4
J?2 ^2 “ P3 > Pl ^1 ” P4 ^4
Define n by V3  n V2
Then p3 = p2n~1 so
P4F4-P3F3-P2 v2n ~y~Pivin ~У
P^l’P^i so V41’T-Vi1-7»1"’ or
f4-
Also
f2
ei-p2F2in^ e'2-p3v3
F3 x_r . F3
1 Y-p2v2ln —


2
Finally
T)= l-^= l-n1-7» 0-242
Й1
p?
(b) Define n by p3- —
P2F27-^F’ or V3- n^V2
So we get the formulae here by n -* ni/y in the previous case.
.	UA)-1	4
r| « 1 - п « 1 - и 7 - 048
2.115
Used as a refrigerator, the refrigerating efficiency of a heat engine is given by
Q2 Q2 Q^Qi 1 -rj
e « —— = —----— = -----—- = -----L = 9 here,
2i ~ Q2 2г Л
'2?
where T) is the efficiency of the heat engine.
228
2.116
Given V2 = n V!, V4 - n V3
2i = Heat taken at the upper temperature
» RTj^ In n + R T2 In n - R(T1 + T2) In n i
/ТЛг-i or V3- / V2 \2)
1 /ту-1 v6- kr Vi I J3I
Now TiF^"1- t2f;_1
1
/Ту-1
Similarly V5 = — V4, Из)
у
Thus Q2 я heat ejected at the lower temperature  - RT3 In — 1 1 ZTAv-iK , /ТЛ?-1 = -ЯТ31п/	-^--ЯТ31п/
У1!) V4	^V3
1 1 /ту-1 1 /ТЛ“7‘ - -/min hr	Лкг
3 nJ	„^r2J
2T, T) « 1 - —-—
1 T1 + T2
2R Т31пи
Thus
2.117
Q'2= Cv(T2-T3)^ ^F2(p2-p3)
Qi~ T^yi(Pi-P4)
_	. V2(p2-p3)
Thus	Г) = 1 - —--------
Vi (Pi -pj
On the other hand, 6P1F/ - p2 F2, p3 F27 - p4 F/ also v2 - n Vj
2.118
Thus Pi- p2n\ p4- p3n'1
and r| * 1 - n1-7, with у « — for N2 this is r| =
2i я (^2 “ ^i) > Q i *
„	, Р2(^-У4)
Р2(Уз-^)
Nowpj- np2, p{ V} or V3
1
nyV2
1 i	—-1
so n = 1 — • ny = 1 - Л 7
229
2.119 Since the absolute temperature of the gas rises n times both in the isochoric heating and in the isobaric expansion
Pi = nP2 and ^2 = n • ^eat taken is
6i - би + 212
where Qn = Cp (n - 1) T\ and g12 « Cv 7\ ^1 - ± Heat rejected is
2'2 “ 2'21 + 2'22 where
e'21- CyT^n-l), Q'^-
Q! Cv(n-l) + cJ И I
Thus T] = 1 — —- « 1 -
21
C/n-l) + CJl-i
° 1"------- V Л ° 1 *
Y (n - 1) + 11 - - j
I
»_ 1	” + Y
1	1 +лу
2.120 (a) Here p2 - np,, p, V, - p0 Vo, »Pi Vl - p0 VJ yo
е'2=ят01п^, 21= CvT0(n-l)
-1
But nV/-1- VJ-1 or, Vi - Vonv-i
Q'-} = RTninn'i~1 - —2-lnn
2 u	y-l
Thus T] =
1-
In n .
-—-, on using Cv »
R Y-l
(b) Here V2- nV,, Р1 V, - Po Vo
and p, (n V,y - p0 VJ
230
2.121 Here the isothermal process proceeds at the maximum temperature instead of at the minimum temperature of the cycle as in 2.120.
(a) Herepx = p0V0, p2 = —
Pl V1 ж Po Vo or Pi Vl ~ np0 Vj
i.e.
Vp'-nVp1 or V,- Von^
I	П	Vi Л7о
e'2- CVTO 1-- , 21» ЯГ01п-^- —ylnn- CvT0\nn-I	П)	Voy— 1
_	. 6'2	< и -1
Thus	Y] - 1 - 77- s 1 - -
1 2i л In л
(b) Here V2-	p0V^-P1V,
PoV^PiVl-Pi^Vl	or V1-n^-i}Vo
Q'i-	Ci= A7oln^=	CpTolnn
n - 1
Thus	r| ® 1 - —:-
1 и In n
P°^°Q, isothermal
PttVhTo
2.122 The section from Vp To) to (p2, V2, T^/n) is a polytropic process of index a. We shall assume that the corresponding specific heat C is + ve.
Here, dQ = CdT = CvdT+pdV
NowpVa« constant or 7Va-1« constant.
„z RT R
so paV = —dV= --------dl
г V a -1

adiabatic
РгУг^Го/п
ЯТ0 P1V, We have P1 Vr« RT0^ P2V2 -
Po V0 - P1V1~ nPl V2> Po V0 - P2 V2>
231
PiVi-PiVi or	or V2-V0nTTT
Vf"1-ly»-1 or V!-и’ТЛУ2- и^Т~^ТУ0
Now Q'2 «
СТо(1-Ч 21-ЛТ01п^-ж ЯТор—-—Ц-)lnn = СТ01пл и1 п) 1 и Vo и1у-1а-11	°
(a)	Here Q'2 - Ср - Ср Т, (1 - Q, - Cv (то -Along the adiabatic line
roVoT_1- Л(«Уо)У-1 or, То-Л”7'1'
so	- Cv-^^n'^ - 1). Thus TJ - 1 -
(b)	Here C'2 - Cv(n Tx - To) ,Qr-Cp-T^n- 1)
Along the adiabatic line TV7 -1 - constant
/yf1
«г T^n^T.
232
(a) Q*2 “ Ср ^0
So
1-л)’ С"1= ЯГо,пи> е'1“
Qi~ Q'i + Q'\
С f1-") w4-i--zy G1 Cv|l-—|+Я1пл I n\
____Y t Y(w-l) R nlnw n - 1 + (y - 1) л In n
+ Cv n - 1
(b) 21 = CP Тй (« - 1), Q"2 - cv To (n - 1) Q"2 -RT0\nn,Q'2- Q"2 + Q'"2
So
2'2 - _ n - 1 + (y - 1) In и
2i“ 	Y(»-1)
2.125 We have
2 1 = •^Y’q in v, 2 2 = С у Yq (t — 1) 2i = Q 1 + 2 1 and 2"'2= /?rolnv, 2”!= Cvr0(T-l)
as well as 2i ж Qi + Q” and
22'= 22" + 22"'
o л Q'2 л Cv(t - 1)+K In v
°	2i + Cv (т - 1) + t R In v
_ 1 _ Y-1 ж (т- 1)Inv
T - 1	X - 1
---- + X In V x hi v +--
Y-1	Y-1
2.126 Here 2/' = CpT0 (r -1), 2'1" = тКТупл and
22" = C%(t-1), 22"'-ЛТ01пл in addition to we have 2i = 2i' + 2i" and
Q2-Q2+Q2"
_ _ 2'2 _ Ср(т- 1) + Я1пи
2i Cp (т - 1) +тЯ1п n
т-1+1-— т1пл l У
In n
x - 1 + I I
1	1 fl 11 1
T-1+1-— Tlnn
I Y
(т - 1) In n
x In n + У
Y-1
233
2.127	Because of the linearity of the section В C whose equation is
^(-P- aV)
Po vo t	r~
We have ~ = v or v  Vt v
Here 6"2- C„TO(V7-1), 2"'2- С,ТО(1--Ц- c,-^(VT-i)
I Vt Vt
Thus Q'2 - Q"2 + Q'"2 - —(V? - 1) 1 + -У Y -1 I Vt )
Along BC, the specific heat C is given by
/1 I 1 \
CdT = CvdT+pdV = CvdT+d -a V2 = Cv + -fl dT
у + 1 т - 1
Thus
2i=
Y-l
Finally
T|»
1-2
Vt +1 Y + l
(y-1)(V7-1) (y+l)(Vr+1)
2
2
2.128	We write Claussius inequality in the form
where dQ is the heat transeferred to the system but d2Q is heat rejected by the system, both are +ve and this explains the minus sign before <t2 Q, In this inequality Tmax > T> and we can write

Thus
^min Ql
0Г T < Qy max
or
1 Q'2 1 _ ^mln ~Ql<
carnot
n =
2.129	We consider an infinitesimal carnot cycle with isothermal process at temperatures T + dT and T.
Let 6A be the work done in the cycle and 6(2, be the heat received at the higher temperature. Then by Carnot’s theorem
234
2.130	(a) In an isochoric process the entropy change will be
/CydT	Tf
—-—= Cv In — = Cv Inn
T
For carbon dioxide y= 1*30
so,	AS = 19-2 Joule/°K - mole
(b) For an isobaric process,
ac i Tf i Y^lnn
AS = C In 77» C In n « J-—
p Tt p ' Y-l
« 25 Joule/^K - mole
2.131	In an isothermal expansion
AS= vRln^
y f AS/VR л so, c = 20 times
2.132	The entropy change depends on the final & initial states only, so we can calculate it directly along the isotherm, it is AS = 2R In n  20 J/*K (assuming that the final volume is n times the inital volume)
2.133	If the initial temperature is To and volume is VQ then in adiabatic expansion,
TV1'1* To Vo’1
у
so,	T = T0n1-7 = T\ where n » -=^
4)
being the volume at the end of the adiabatic process. There is no entropy change in this process. Next the gas is compressed isobarically and the net entropy change is
235
But
Vi v0 T Tv0 T
T^’ or Tf- T1?-- Ton
C	AC	1	m 1	m R4 	niT/V
So	AS- —C„ In — «	- —C„lnw-	-77—*— In и -	-9-7J/K
\M fj n MP My-I
2.134
The entropy change depends on the initial and final state only so can be calculated for any process whatsoever.
We choose to evaluate the entropy change along the pair of lines shown above. Then ro	q7o
₽	₽
Г vCydT Г dr I т +J vCp T  \
= (- Cvln p + Cp In a) v - (y In a - In P) •» - 11
To calculate the required entropy difference we only have to calculate the entropy difference for a process in which the state of the gas in vessel 1 is changed to that in vessel 2.
0
AS-
2.135
2.136
Po,Vq7o
T-’v^
«₽
I rl
«₽
« v (Cp In a - Cvln оф)
- v (a In a - —In = v7?(lna-^-^-
l Y-1 /	\	Y“1
With y= |, a = 2 and p= 1-5,v= 1-2,
AS- v
this gives AS « 0-85 Joule/°K
i t Tt
For the polytropic process with index n pVn - constant
Along this process (See 2.122) r 1	1	”~Y
C'R	(Y-i)(„-i) *
So
J Cf= (У-'1‘)(п-1)Л1ПХ
T„


v
236
2.137	The process in question may be written as
P	У
Po	Уо
where a is a constant and p0, Vo are some reference values. For this process (see 2.127) the specific heat is
C= С„+|к= /?[-Ц- + Д =
v 2	I у -1 21 2 у-I
Along the line volume increases a times then so does the pressure. The temperature must then increase a times. Thus
A
AS- f ^^Ц-lna2- у In a
J T 2 Y-l	Y-l
r0 '
if	v - 2, y - a = 2, Д5 - 46-1 Joule/°K
2.138	Let (pv VJ be a reference point on the line P = Po - aV and let (p, V) be any other point. The entropy difference
AS- S(p,V)-5(p1,V1)
p V Po~aV V
- Cy In—+C In-;?-- Cvln------+ С-1П-1-
Pi p ?i	Pi p vi
For an exetremum of AS
dAS _ ~aCy	Cp
dV Po~aV+ V ~
or Cp (p0 - a V) - a VCv- 0
YPo
or Y(P0-aV)-aV- 0 or V- Vm- -°-°	"* a (y + 1)
^2
This gives a maximum of AS because-у < 0
dV2
(Note a maximum of AS is a maximum of S (p, V))
2.139	Along the process line : S « aT + Cv In T dS or the specific heat is : С « T « aT + Cv
On the other hand : dQ « CdT « CvdT+pdV for an ideal gas.
Thus,	pdV-	dTdT
237
or	—— « dT or, — In V + constant = T
a V	a
R V
Using T  TQ when V = VQ, we get, T = TQ + — In —
a *0
2.140	For a Vander Waal gas
The entropy change along an isotherm can be calculated from
It follows from (2.129) that /а$\ (dp\ R (av)rs v-b
assuming a, b to be known constants.
Thus	AS-In-^—7-
assuming CVt atb to be known constants.
2.142	We can take S -* 0 as T -* 0 Then т	т
s-J*Cy~J*aT2 dT,‘ 5ат3 о	0
т2 тг
2.143	AS.	f W (ay № dT - mb (Г2 - Tr) + ma in
238
2.144	Неге Т= aSn
Then
Clearly
2.145 We know, т
assuming C to be a known constant.
/5-50'
Then T« Toexp I———
2-146 (a) C= v ' dT T
T2
CdT- alnzr 72 Г
(c) 1¥== A£ - At/ = a In zr + Cy(T\ - T2) 22
Since for an ideal gas Cv is constant and At/=
(U does not depend on V)
2.147 (a) We have from the definition
Q = J* TdS = area under the curve
2i= Tq (Sj - s0)
e'2- |(ro+r1)(s1-so)
To
Thus, using 7j = —
1 1
. To+ Ti	,	+ n	n-l
1	2 To	2	2n
(b) Here Ci - |(51-50)(T1 + 7’0)
Q ' 2 “ ^1 (^1 ~ ^o)
2Tt	n-l
П‘ + То-Т\~ n + 1
239
2.148
In this case, called free expansion no work is done and no heat is exchanged. So internal eneigy must remain unchanged Uj « Ц. For an ideal gas this implies constant temperature Ту « T? The process is irreversible but the entropy change can be calculated by considering a reversible isothermal process. Then, as before
V2
vR\nn = 20-1 J/K

2.149
The process consists of two parts. The first part is free expansion in which Uj « Ц. The second part is adiabatic compression in which work done results in change of internal energy. Obviously,
0= UF-Uf +f pdV, Vf =
2V0
Now in the first part pf »
1 rz 2Po> Vf“
2Vg, because there is no change of temperature.
In the second part, p VY -	(2V0)Y - 2 Y ~ гр0 Vj
-Y + l
/I 2Y_1poVoY J ------------
2Vo 2V0 ,	i 2-Y + 1-1	(2Y-1-1
- 2Y-1Po^ V+1 Чтт-1- -
^0
Y-l
Thus
R T &u= Up-Ui = ^(2Y-1-i)
The entropy change AS = ASZ + ASn
2.150
ASj» К In 2 and Д5Я = 0 as the process is reversible adiabatic. Thus AS = К In 2.
In all adiabatic processes 2= С/ГЦ+А= 0 by virtue of the first law of thermodynamics. Thus,
Uf = Ui-A v
For a slow process, A' = J* pdV where for a quasistatic adiabatic process pVy « constant. vo
On the other hand for a fast process the external work done is A” <Af, In fact A" « 0 for free expansion. Thus U'j (slow) <U"j (fast)
Since U depends on temperature only, Гу < T'y
Consequently, p" >p'j
(From the ideal gas equation pV = RT )
240
2.151 Let - Vo, V2 - n VQ
Since the temperature is the same, the required entropy change can be calculated by considering isothermal expansion of the gas in either parts into the whole vessel.
Vr + v2 Vr + v2
Thus A5= AS^ + AS^» v^ln—+ v2T?ln———
V1	V2
- v^lnQ + л) + у2Л1п^-^ - 51J/K
2.152 Let cr « specific heat of copper specific heat of water = c2 tq	97 + 273
/c2m2dT | mrcrdT	TQ	370
T J T 2 280	1 1 TQ
7 + 273	To
TQ is found from
280 m2 c2 + 370 m, Ci c2m2 (To - 280) - m. q (370 - To) or To - -r	1
c2 m2 + mY cr
using	cT - 0-39 J/g °K, c2 - 418 J/g °K,
2.153
2.154
To ~ 300°K and AS » 28-4 - 24-5 « 3-9 J / °K
For an ideal gas the internal eneigy depends on temperature only. We can consider the process in question to be one of simultaneous free expansion. Then the total energy U = Ur + U2. Since
Г +^2
Ux = CvTlf U2 = CVT2, U= 2CV—-— and (Тг + Т2)/2 is the final temperature. The entropy change is obtained by considering isochoric processes because in effect, the gas remains confined to its vessel.
(Tt + T2)/2
2
AS»
/dT (Ti + T2)2
C ^L_ C In *	2
v T v 4T1T2 (rl+T2)/2
Since
(Л + Ту2- (T1-T2)2 + 4T1T2, Д5 > 0
(a)	Each atom has a probability to be in either campartment Thus
P 2
(b)	Typical atomic velocity at room temperature is^ 105 cm/s so it takes an atom 10~5 sec to cross the vessel. This is the relevant time scale for our problem. Let T = 10 ” 5 sec, then in time t there will be t/T crossing or arrangements of the atoms. This will be large enough to produce the given arrangement if
— 2~N ~ 1 or	~ 75
т	In 2
241
2.155 The statistical weight is
. T	N\ 10 x 9 x 8 x 7 x 6 ___
Nr " -----ГГ" —о—1;—z—» 252
c*/i JV/2 i — i	8 x 4 x 3 x 2
’ 2*
2.156
The probability distribution is
Nc 2~n - 252 x 2“10- 24-6 %
The probabilites that the Jialf A contains n molecules is
wc X	2^
ся	n ! (N - n) !
2.157	The probability of one molecule being confined to the marked volume is
We can choose this molecule in many (Nc ) ways. The probability that n molecules get confined to the marked volume is cearly
NeP’ll-pf-’-
2.158	In a sphere of diameter d there are
N = ”o molecule
where nQ = Loschmidt’s number = No. of molecules per unit volume (1 cc) under NTP.
The relative fluctuation in this number is av Vn i N ~ N “ Vy ” Л
.1/3
or	or d3»—or d« |—~—I	= 0-41 цт
г] о	л nQ rf	I я Л п0 J
The average number of molecules in this sphere is « 106
3
2.159	For a monoatomic gas Cv« — R per mole
The entropy change in the process is Т0 + ДТ
AS-S-S0 = J CFy-+^r|
To
Now from the Boltzmann equation
S- ilnQ
3x6од»
А+АГ)2 .A	- io13 x io21
Qo	I Tq I	300j
Thus the statistical weight increases by this factor.
242
2.5 LIQUIDS. CAPILLARY EFFECTS
2.160
*	( 1	1 \ 4a
Ap~ a[d/2 + d/2)~ d
 --X	x 10—1-307 x 10 6	13 atmosphere
1-5 x IO-6 m2	m2
(b) The soap bubble has two surfaces л i ( 1	1 \ 8a
so	^'2а(572^)-Т
*>	x 10~3 - 1-2x 10 “3 atomsphere.
3xlO"3	r
2.161 The pressure just inside the hole will be less than the outside pressure by 4 а/d. This can support a height h of Hg where
. 4а . 4а pgh- — or Л = ------
d	pgd
4 x 490xl0’3	200
« ------5----------z - ———— - -21 m of Hg
13-6 X 103 X 9-8 X 70 X 10  6	136 x 70
2.162 By Boyle’s law
/	8а \ 4 л (d\ [Po 8а | 4л /т] d\
P+ d) 3 I2J “ +na) 3 2 J
(, n3^ 8а. 2 .X
1- л I” -1)
Thus	а - |p0 d ^1 - (tj2 - 1)
2.163 The pressure has terms due to hydrostatic pressure and capillarity and they add 4а
P- Po + P8h + ~[-
(. 5X9-8X103 4x-73xl0-3 .„-s'! .	.
= 1 +-------<--+-----------7— x 10	atoms « 2-22 atom.
10s	4xl0’6	J
2.164 By Boyle’s law
L ь 4а\ яд (	4а\ я з л
or	pigp -Ро (л3 - 1)] -	(«2 - 1)
or
Ро(л3-1) +	(л2-1) /gp
в 4-98 meter of water

243
2.165	Clearly
АЛ p g « 4 a | cos 0 11 -y- - -y-\ 1
4a|cos0|(d2-d1)
ДЛ « -----—----------- = 11 ]
2.166	In a capillary with diameter d = 0-5 mm water will rise to a height
2a 4a
PS'" pgd
4x73xl0“3
—z-------------7= 59-6 mm
103 x 9*8 x 0-5 x 10“3
Since this is greater than the height ( = 25 mm) of the tube, a meniscus of radius R will be formed at the top of the tube, where
_ 2a 2x73xl0-3
R = ---- = —z----------т * 0-6 mm
Pgh 103 x 9-8 x 25 x 10 -3
2.167	Initially the pressure of air in the cppillary is pQ and it’s length is I. When submerged under water, the pressure of air in the portion above water must be pQ + 4~, since the level of water inside the capillary is the same as the level outside. Thus by Boyle’s law
^Po + ^(z-x)“ Pol
4a	I
or	— (l-x)-pox or X=—
1+ 4a
2.168
We have by Boyle’s law I . 4acos0\/f M , |Po “ Wh +------------2--- (Z “ Л) = Po1
4 a cos 0	, Pq h
——
1ря'‘*г-л1то„;'о
or,
Hence,
2.169
Suppose the liquid rises to a height h. Then the total energy of the liquid in the capillary is
^(^-d^hxpgx^-nid^-d^ah
Minimising E we get
, 4a £
h = —71—7T = & Pg (d2 - dj
244
2.170
dx • h • a cos 0
dx^-xpgbtp
2.171
Let h be the height of the water level at a distance x from the edge. Then the total energy of water in the wedge above the level outside is.
E-§ x b <p • dx • h • P^2
1	c /,2 ~ 2 a cos 0 . \ ar—xpgocp\h -2--------z—h\
2	I xpgbcp J
2 \	2 a cos 0\	4 a2 cos2 0
k	* P g 6 qj	x2 p2 g2 6 ф2
.	• • u 1.	2 a cos 0
This is minimum when h  ---;—
xpgbtp From the equation of continuity зг j2	/a 1 тг тг 2
— a • v = ~ - • V or V = n v.
4	4 In I
We then apply Bernoulli’s theorem p 1 7 *- + — v + Ф = constant P 2
The pressure p differs from the atmospheric pressure by capillary effects. At the upper section
2a p~p^~a
neglecting the curvature in the vertical plane. Thus, 2a	2na
P"*7 1 2	, ’’•>*— 1 . 2
-Г" T *«'-------—*2" v
or
v =

n4- 1
Finally, the liquid coming out per second is,
4a
n-l
2.172 The radius of curvature of the drop is R{ at the upper end of the drop and R2 at the lower end. Then the pressure inside the drop is pQ + at the top end and pQ + at the bottom Ki	R2
end. Hence 2a 2a , P° + Po + ^+Pgfl
or
To a first approximation Rr ** R2 *
so
2a(R2-Rt)
~R^-
1	3
— pg/r/a. « 0-20 mm о
h - 2*3 mm, a = 73 mN/m
246
2.173
We must first calculate the pressure difference inside the film from that outside. This is
/1 p  a — + — I.
V1 M
Here 2 rr |cos 0 |« h and r2 ~ - R the radius of the tablet and can be neglected. Thus the total force exerted by mercury drop on the upper glass plate is
2 л R2 alcosOl .
--------------1 typically
We should put h / n for h because the tablet is compresed n times. Then since Hg is nearly, incompressible, jeR2 h = constants so R -> R>/n . Thus,
* - r 2 л R 2 a |cos 0 I 2
total force « --------H-------1 n
h
Part of the force is needed to keep the Hg in the shape of a table rather than in the shape of infinitely thin sheet. This part can be calculated being putting n «1 above. Thus
2 л R2 a I cos 01	2 л R2 a I cos 0 I 2
mg +----------J------d = ---------J-------1 n
2.174
2.175
2.176
h	h
. 0.7k
The pressure inside the film is less than that outside by an amount a | — 4- — | where \ 1 Г2)
rr and r2 are the principal radii of curvature of the meniscus. One of these is small being given by 2rxcos0 while the other is large and will be ignored. Then
2 A cos 0
F« ---------a where A « area of the water film between the plates.
Now A = so F - when 0 (the angle of contact) = 0
This is analogous to the previous problem except that : A = л Я 2 2я^2? ж 0-6 kN h
The energy of the liquid between the plates is h
£= ldhpg^-2alh~ ±pgldh2-2alh
1	, j (,	2 a \2 2 a2/
= rpg/« In-------- --------
2	5 pgd] pgd
This energy is minimum when, h « --7 and
2 a2 I the minimum potential eneigy is then Emin = - — 
The force of attraction between the plates can be obtained from this as F = ——77^ ж ~	1 (minus sign means the force is attractive.)
dd pg^T
or
So
Thus
m =
	.•‘J П1Г l(| P| z'1 i'!' 1 i'K	h
Hl Hl III	1И!1 :: j_lL		1» 	—
'I1' P1! 11M (III	li(l’ '[ill	— —		
——• —•	L — "		—~ -7— -
F=	13N
a
246
2.177
Suppose the radius of the bubble is x at some instant. Then the pressure inside is 4cx
Po + —. The flow through the capillary is by Poiseuille’s equation,
C-
лг4 4a
8т) I x
- 4я
2 dx dt
т _ яга Integrating -y^-
я (R4 -x4) where we have used the fact that t •
0 where x « R.
2 n IR*
This gives t« —L-r— as the life time of the bubble corresponding to x = 0 ar
2.178 If the liquid rises to a height Л, the energy of the liquid column becomes 2	2
£ pen^h-^-lnrha.* тр£л[гЛ-2-^-| -	- -
2	2HS I pgl pg
2 a	'	'
This is minimum when rh - -and that is relevant height to which water must rise.
Pg	2
At this point,	£min  - -	
2 л a2 Since E « 0 in the absence of surface tension a heat Q  -must have been liberated.
Pg
2.179 (a) The free eneigy per unit area being a, я a d2 « 3 pJ
(b) F~ 2 я ad2 because the soap bubble has two surfaces. Substitution gives F - 10 pl
2.180 When two mercury drops each of diameter d merge, the resulting drop has diameter dt where	« ~d3 x 2 or, dt« 21/3 d
The increase in free energy is
AF« n22/3d2a-2nd2a^ 2nd2a (2-уз - 1) - -l-43pJ
2.181 Work must be done to stretch the soap film and compress the air inside. The former is simply 2 a x 4 яR2  8 яЛ2a, there being two sides of the film. To get the latter we note that the compression is isothermal and work done is
vf-v
- J* pdV where VoPo' (ро + ‘уИ’у> ^r3
V,- vo	'	'
v pV 4 a
or	Vo~ n’p~ P° + ~R~
Pq	л
and minus sign is needed becaue we are calculating work done on the system. Thus since pV remains constants, the work done is
VQ	P
pVln-£-pVln£-
V	Pq
So	A' « 8яЯ2а+р Vln —
Pq
247
2.182 When heat is given to a soap bubble the temperature of the air inside rises and the bubble expands but unless the bubble bursts, the amount of air inside does not change. Further we shall neglect the variation of the surface tension with temperature. Then from the gas equations
(4 fi\ 4 тг i
p0 +--- — r3 « vRT, v = Constant
u r I 3
Differentiating
+ 3>r j 4 л r2 dr = vRdT
a 2 j v/?JT
or	dV = 4лг dr = ------—
8
Now from the first law
. _	~ 1r_ vRdT
d" Q* v CdT = v CvdT +------—
v	8a
4a
Po + ~
or	C= Cv + R----—
v 8a
*>з7
2
using	Cp~ Cv + R, C-Cp +------r
i+~sT
2.183
Consider an infinitesimal Carnot cycle with isotherms at T - dT and T. Let A be the work done during the cycle. Then
A- [a(T-<fl)-a(7)]&0- ~^dTba Where ba is the change in the area of film (we are considering only one surface).
A dT
Then Ti = — = — by Carnot therom. 1	Ci T 3
-^dTba	,
dT	dT	„ da
or-----x----= V or 4 = " 37
q 0 a T	dT
2.184
As before we can calculate the heat required. It, is taking into account two sides of the soap film
Thus
bq- -Td^ox2
bq	da Q
AS= -^-= -2—ba
T	dT
Now
AF = 2 a b a so,
AF + TAS-
248
2.6 PHASE TRANSFORMATIONS
2.185	The condensation takes place at constant pressure and temperature and the work done is pA V
where AV is the volume of the condensed vapour in the vapour phase. It is
pM'* ~RT = 120-6 J M
where M - 18 gm is the molecular weight of water.
2.186	The specific volume of water (the liquid) will be written as V? Since Vv > > V\y most of the weight is due to water. Thus if ml is mass of the liquid and mv that of the vapour then m =	+ mv
V-mlVl + mvVv or V-mV, = mv (V„ - Vt)
So
V-mV, Vv-Vi'
20 gm in the present case. Its volume is mv Vv « 1-01
2.187	The volume of the condensed vapour was originally Vo-V at temperature T « 373 K.
Its mass will be given by
/Т7 ТЛ m n-r	MP (^0 - V) „
P i'o - П	or m - ------—-------= 2 gm where p -
1x1
atmospheric pressure
2.188	We let Vz« specific volume of liquid. Vv = N * specific volume of vapour.
Let V = Original volume of the vapour. Then pV	V V
MRf" mi + mv- хуг or (mt+Nmv)Vt
(1\ V	miV,	n-i
or	——
n\n	V/n	N -1
In the case when the final volume of the substance corresponds to the midpoint of a horizontal portion of the isothermal line in the pt v diagram, the final volume must be
(1 + N) — per unit mass of the substance. Of this the volume of the liquid is У\/2 per unit total mass of the substance.
n,»	Ч'гЪ
2.189	From the first law of thermodynamics
AL7+A = Q = m q where q is the specific latent heat of vaporization
Now	A = p (Vv~V^m =
M
Thus	AU = m(q- —y)
I Ml
For water this gives « 2-08 x 106 Joules.
249
2.190	Some of the heat used in heating water to the boiling temperature
T = 100°C = 373 К The remaining heat
« Q-mcAT
(c - specific heat of water, AT» 100 K) is used to create vapour. If the piston rises to a height h then the volume of vapour will be - 5A(neglecting water). Its mass will be pQsh	p^shMq
x M and heat of vapourization will be ———. To this must be added the work KI	KT
done in creating the saturated vapour * posh. Thus
Q-mcAT - p0Sh [1 + or A -	« 20 cm
1 QI	~ ^0)
a quantity ----------of saturated vapour must condense to heat the water to boiling
point T= 373°K
(Here c  specific heat of water, To « 295 К « initial water temperature).
The work done in lowering the piston will then be
q M '
RT
since work done per unit mass of the condensed vapour is p V  —
2.192
or
— ЛТ
An Pv2a Pv 4a	M x]RT
Given ДР- — — - — x —- Tt\p - T]——- -^з-р, Pl ? Pl &	'vap M
4aM
Pi^4
For water a • 73 dynes/cm, M« ISgm, p; - gm/cc, T» 300 K, and with r) < 0 01, we get d- 0-2pm
2.193 In equilibrium the number of "liquid" molecules evaporating must equals the number of "vapour" molecules condensing. By kinetic theory, this number is
1	1	, /в kT
ri x — n <v > - Ti x — л x v-
1 4	4 ’am
Its mass is
------- ri nkT v -—r 2nm 1	* 2nkt
° ^NI^rt ’ 035 g/cm2 s-
where pQ is atmospheric pressure and T = 373 К and M = molecular weight of water.
250
2.194 Here we must assume that p, is also the rate at which the tungsten filament loses mass when in an atmosphere of its own vapour at this temperature and that r] (of the previous problem) 1. Then
И

M
= 0-9 nPa
from the previous problem where p = pressure of the saturated vapour.
2.195
From the Vander Waals equation RT a
P~ V-b V2
where V Volume of one gm mole of the substances.
For water V * 18 c.c. per mole  1-8 x 10 “ ^itre per mole _	litre2
a = 5-47 atmos-------г
mole
If molecular attraction vanished the equation will be
, RT P “ V-b
for the same specific volume. Thus
Ap «	x 104 atmos - 1-7 x 104 atmos
V2 1-8 x 1-8
2.196	The internal pressure being the work done in condensation is
v g
J v2 vt v vt
v,
This by assumption is Mq, M being the molecular weight and Vt, Vg being the molar volumes of the liquid and gas.
™	fl Mq
Thus
where p is the density of the liquid. For water  3-3 x 1013 atm
2.197	The Vandar Waal’s equation can be written as (for one mole)
At the critical point МЫ and vanish. Thus Wt Jr
n RT	2a RT	2a
О ш -----у + —т ОГ ------у я —у
(V-b)2 V*	(V-b)2 V3
л 2RT	6 а RT	За
О = -----Г---Г ОГ -------Т “ —л
(V-b)3 V4	(V-b)3 V4
251
2.198
2.199
2.200
Solving these simultaneously we get on division
v-b-|v, V= 3b  VMCr
This is the critical molar volume. Putting this back
R Per 2a т Qa 01 Cr= 27 Ы?
M	^cr a 4a a a
Ша У Pcr~ VMCr-b vic" 27 b2 9 b2" 27 b2
,	^сг^мсг a/9b 3
From these we see that	——-----= -—= ~~
RTCr 8a/27 b 8
Per _ a/Xlb2 _ 1
RTCr 8 a/21 b 8 b
-to.	. D ?cr 0 082x304
Thus	b = R ----= ———-— = 0 043 litre/mol
8 pCr	73 x 8
A (RTcr)2 64a	(Огг X2Z - CQ atm-litre2
and -----------= or a = — (RT) /p = 3-59---------------z—
Per	6* Cr Cr	(mol)2
Specific volume is molar volume divided by molecular weight. Thus
„	VMCr 3RTcr 3x-082 x 562 litre cc
Cr“ M “ 8MpCr“ 8x78x47 g " g
'p + -^\(VM-b)=RT
I M) a p + —^
Vm Vm-b 8 T or	—=:--x -----= z-
Per VMCr 3 TCr (	« W \	8
or	Л +--о X V-—---- « — T,
(	PcrV^j I	M	3
.	p	T
where	л = —, v= ——, t= —
Per VMCr TCr
2.201
13	13
When	л  12 and v  —, т  - x 24 x - « —
2	8 о 2
(a)	The ciritical Volume VMCr is the maximum volume in the liquid phase and the minimum volume in the gaseous. Thus
X 3 X -030 litre - 5 litre
252
(b)	The critical pressure is the maximum possible pressure in the vapour phase in equilibrium with liquid phase. Thus
27 x «’к <B ’
2.202	= 27M “ 27 х -043 x-082 “ 3O4K
M 44
pCr- — - ^-^-gm/c.c. - 0-34gm/c.c.
2.203 The vessel is such that either vapour or liquid of mass m occupies it at critical point. Then its volume will be
_ HLV _ 3 RTC'm
Vcr ~ M MCr ~ 8 pCr M
The corresponding volume in liquid phase at room temperature is
2.204
y= ™ P
where p = density of liquid ether at room tmeperature. Thus
у 8MpCr
0-254
3 RTCr p using the given data (and p « 720gm per litre) We apply the relation (T« constant)
T<f dS-f dU+f ;
to the cycle 1234531.
Here f d$-(f dU-0
So
j pdV =0
This implies that the areas I and II are equal. This reasoning is inapplicable to the cycle 1231, for example. This cycle is irreversible because it involves the irreversible transition from a single phase to a two-phase state at the point 3.
2.205 When a portion of supercool water turns into ice some heat is liberated, which should heat it upto ice point. Neglecting the variation of specific heat of water, the fraction of water turning inot ice is clearly
f =	0-25
4
where c== specific heat of water and q - latent heat of fusion of ice, Clearly f = 1 at t - - 80“C
253
2.206 From the Claussius-Clapeyron (C-C)equations
dT 4p "	«12
g12is the specific latent heat absorbed in 1 -* 2 (1 = solid, 2 = liquid)
273 x ,091 atm x cm3 x К
A T « — ----------Ap « ------—------x 1-----:—;------
qn	333	joule
3	105-^xl0’em3
1 atoa><cm ------------------» 10 -1, AT------0075 К
Joule	Joule
2.207 Here 1 = liquid, 2 = Steam
«12
or

«12 AT= 2250
T bp 373
x j^xl0-3m3/g = 1-7 m3/kg
2.208 From C-C equations
dp	#12	#12
df~ T(V2-V^ TV~2
Assuming the saturated vapour to be ideal gas
~T= Thus Ap = ^^pAT
V2 RT	RT2
and
p » poll + A T| л 1-04 atmosphere I RT I
2.209 From C-C equation, neglecting the voolume of the liquid
or
Now
So
dp #12
dT “ TV2
« ^P, (« - «12)
dp Mq dT p Ж RT T
pV« ;RT or m  for a perfect gas M	KT
- —7 (V is Const = specific volume)
RT I T 8-3x373 I 373
264
2210
From C-C equation
Integrating In p
dp q Mg
dT~ TV2" rT2P
t . мч constant -RT
Mg_(l_ IV r ko~Tl
This is reasonable for |T - To|« To, and far below critical temperature.
So
p-pocxP
2.211
As before (2.206) the lowering of melting point is given by
.„ Г AV AT = ------p
The superheated ice will then melt in part The fraction that will melt is
CT AV
П - ---2—P ~ ’°3
9
2212
(a) The equations of the transition lines are
,	1800 „ ...
log p » 9-05-----—: Solid gas
1310 .. .. - 6-78 - у : Liquid gas
At the triple point they intersect Thus 490	490
2-27= or 7, = — - 216K
corresponding p№ is 5.14 atmosphere.
In the formula log p= a we compare b with the corresponding term in the equation in 2.210. Then
i	о ini 2-303 b c _ ,n- M<j
In p  a x 2-303 - —у— So, 2-303 -
or,
2-303x1800x8-31	7„T,
QsMimation = -----^4---------- 783 J/gm
2-303 x 1310 x 8-31	,_n T .
---------- 57°J/gm
Finally qmlid_14uiji- 213 J/gm on subtraction
T2
2.213 .„ f dT mg ( . ^2 q\
“-J •‘rt- Kt
Tt	'	'
и’(ф18,"В+^-7:!Ы/к
255
2.214 AS» ^ + cln-F+^
Tr T T2
2.215
2.216
333 Л1О1 373
—гг + 4-18 In TTT-
273	283
8-56 J/°K
c » specific heat of copper » 0-39—^—•Suppose all ice does not melt, then g * K
heat rejected = 90 x 0-39 (90 - 0) = 3159 J
heat gained by ice « 50 x 2 09 x 3 + x x 333
Thus	x = 8-5 gm
The hypothesis is correct and final temperature will be T = 273K.
Hence change in entropy of copper piece
273
- me In =£ - - 10 J/K.
363
(a)	Here t2 = 60°C. Suppose the final temperature is t °C. Then heat lost by water = m2 c (t2 -1)
heat gained by ice = qm + c (f - rj , if all ice melts
In this case qm - m2x 4-18 (60 - r), for ?n1» m2
So the final temperature will be 0°C and only some ice will melt.
Then	100 x 4-18 (60) » m\ x 333
m\ « 75-3 gm = amount of ice that will melt
Finally
333	273
AS - 75-3 x |g+ 100 x 4-18 In
Ae '«'l?».	, Tl
+ /П2С1П — 12
T2 - m2 In —
2 Л
Ui-Ti)
» m2c—----
2 Л
= m2c
z ,	. ^2
— -1-In —
8-8 J/K
(b)	If m2 c t2 > qm then all ice will melt as can be obtained like this
m2c(T2-7) = m1qm + m1c(T-T1)
(.m2 T2 + mr Ti) c - qm - («^+ mJ cT
T- -------------— •
m1 + m2
m,q	(	T	T2\
AS- -v1+clm1ln^-m1ln^|. 19J/K
11	I	11	1 I
or
and
one can check and the final temperature

266
то. T2 Mq-
2.217 AS----=H-mcln/ + -^
Z2	T\
where	M qke - m(q2 + c (T2 - Tr)
/ 1	1 \	/2*2	^2^
42 (Л T2j	Tj
» 0-2245 + 0-2564 - 0-48 J/K
2.218	When heat dQ is given to the vapour its temperature will change by dT, pressure by dp and volume by dV> it being assumed that the vapour remains saturated.
Then by C-C equation
f" TV ^vapour >>гь4)> or
RT
on the other hand, pV « —
M
So	pdV+ V dp-^,
M
Hence	pdF- l£-£}dT
Tj
finally	dQ - CdT - dU+pdV'
- CvdT+(£-$\dT- CpdT-^dT I2V2 11	*	1
(Cp, Cv refer to unit mass here). Thus
С- С -Я-p T
For water C =	with y = 1-32 and M* 18
p y-l M ’
So	Cp- l-90J/gmK
and	C - - 413 J/gm°K - - 74 J/mole К
2.219	The required entropy change can be calculated along a process in which the water is heated from TY to T2 and then allowed to evaporate. The entropy change for this is
AS= C,ln^ + ^£
where q » specific latent heat of vaporization.
257
2.7 TRANSPORT PHENOMENA
2220 (a) The fraction of gas molecules which traverses distances exceeding the mean free path without collision is just the probability to traverse the distance s - X without collision.
Thus	P-e"1-—-0-37
e
(b) This probability is
P- e-1-e"2- 0-23
2.221 From the formula
1 -aja i AZ — “ e or л- ;--------
T|	1ПТ)
2.222 (a) Let P (r) « probability of no collision in the interval (0, t). Then
Р(г + Л)- P 0(1-a A)
-aP(t) or P0- e
where we have used J? (0) (b)
or
1
The mean interval between collision is also the mean interval of no collision. Then
2.223
o
kT
1-38 x 10 ~ 23 x 273	„ .„_8
r=-------------sn?-----? - 6-2 x 10 m
2 я (0-37 x IO-9 )2 x 105
X 6-2 x 10-8
Te ----- -----—------5я -136 ns
• <v >	454
6.2 x IO6 m
T)= l-36xl04s =
2.224
X  (b) The mean distance between molecules is of the order
/	a	1Л
(22-4xl0-3\	/224\ in_9 t ... 1n_9 t
-------- s —-	x io meters « 3-34 x 10 meters
6-0 xlO23 I 6 I
3-8 hours
1
7nd2n
0
1 Г (2)	1_
а Г (1) “ a
2.225
This is about 18.5 times smaller than the mean free path calculated in 2.223 (a) above. We know that the Vander Waal’s constant b is four times the molecular volume. Thus / ы \1/3
b~ 4Nat<? or I^TF
6	2 лл.
2/3
Hence
258
2.225 The velocity of sound in N2 is
so,	1-	____
v ’ M y/2nd2pQNA
O',
2227 (,) X>Zif/>< tT v2 шГ1
kT
-- --- for O2 of О is 0-7 Pa.
7 nd2/ 2
(b) The corresponding n is obtained by dividing by kT and is 1-84 x IO20 per m3 « l-8414per c.c. and the corresponding mean distance is ~77v
n~
Now
2.228
10“2
- -------Гл-----x 10 m " 0-18 pm.
(0-184)1/3 x 105
Z 4	1	1	<V>
V т X/<v > X
- V2 n d2n <v > » -74 x IO10 s “1 (see 2.223)
(b) Total number of collisions is
i-nv « 1-0 x 1029 s cm“3
2.229
Note, the factor • When two molecules collide we must not count it twice.
(a) X =	.
V2 я d n
d is a constant and n is a constant for an isochoric process so X is constant for an isochoric process.
V»
87? Г
4^-aVf
A
2.230
(b) X
kT
o — a T for an isobaric process. 'find2 P
<v> УТ 1	.	.
v - —— a ~r- - ~r= for an isobaric process.
X T V7	v
(a)	In an isochoric process X is constant and
kT
(b)	Xs -7=-----must decrease n times in an isothermal process and v must increase
V2itd2p	'
n times because <v > is constant in an isothermal process.
1
269

Thus
7.1/2 к а V and v а
But in an adiabatic process I у -
7VY“1 - constant so TV275 * constant
or	Tw аГи Thus v a Vys
(b)	Ха — P
But p^ “ constant or ~‘аР1/т 01 Tap1”17”
Thus	кар'17’» P'571
1 1+1
<V> P 1/2*2? 2y 6/7
V- _аЛ.ар	p ж
л» VT
(c)	kaV
But	TV2Z5 - constant or VaT~5/2
Thus	Ха T" 5/2
Tl/2
v а у a T3
2.232	In the polytropic process of index n
pVn ” constant, TV"-1« constant and p1-" T"  constant
(a)	ХаУ —1/2	t~"	-л + 1
va^y-- V 2 V1- V 2
(b)	XaJ, T’ap'”1 or Tap1"-
so	кар’1/11
<v> p i_l+-L 1±1 V«—а-7-ар 2 2л«р2я X Vy
(c)	Xa —, pa Г Л”1
1 n 1 1
ХаТ “л1- Г’л-1- Тх"л п 1 я +1 гаДаГ'1'5- Т2^ УТ
260
2.233	(a) The number of collisions between the ^nolecules in a unit volume is
1	1 л2 2 Vf
— -—ltd n <v>a—7
2	V2	V2
This remains constant in the poly process pV~2  constant Using (2.122) the molar specific heat for the polytropic process pVa - constant,
is
Thus
c~ И-Ц-+11-ly-1 41	12 41	4
It can also be written as ^7? (1 + 2 i) where i - 5
p
(b) In this case -y- - constant and so pV~ « constant
so	С-+ Л- + ЗЛ
ly-1 2 J	12 2)
JR
It can also be written as — (< + 1)
2.234 We can assume that all molecules, incident on the hole, leak out. Then,
-dN~ -d(nV)-^n<v>Sdt
or
dn -
dt _ Л n 4v/S <v > П т
Integrating
n - nQ e~t/x. Hence <v > =
2.235 If the temperature of the compartment 2 is times more than that of compartment 1, it must contain — times less number of molecules since pressure must be the same when the big hole is open. If M - mass of the gas in 1 than the mass of the gas in 2 must be M
—. So immediately after the big hole is closed.
о M о 3/
Л. e tz * ^2 я tz 1 mV 2 mVx\
where m  mass of each molecule and n[, are concentrations in 1 and 2. After the big hole is closed the pressures will differ and concentration will become and n2 where M „ X
И1 + n2 « —— (1 + rj)
On the other hand
nl <V1 > * n2 <V2 > П1 ж n2
261
Thus	«2(1+Vn)-^г-(1+т])-^(1+n)
cn	„ _ о 1+П
2.236 We know 1,1	1	./=r
T]- -<V>kp. 7<V>^w^2WaVr °	э V2 ла
Thus T) changing a times implies T changing animes.
On the other hand i л i^/SkF kT D~ — <V>A- — V------------------7=----T““
3	3 ’ am V2nd2p
j3/2
Thus D changing £ times means changing £ times
о	v a3 .
So p must change — times P
2.237 Da — <*VVT, n«VF n
(a)	D will increase n times
r) will remain constant if T is constant
*p3/2	/ ▼>\3/2
(b)	Да —-p^y372 P P
T] a VpV
Thus D will increase л3/2 times, r| will increase n1/2 times, if p is constant
2.238 z>a vVt , T]aVf
In an adiabatic process
TV*”1« constant, or Ta V1-7'
Now V is decreased — times. Thus n
2.239
xZJL 4/5
3-Y	/]\	2	/1\
DaV2 - -	« Г
l n I	\n I
1-Y	/чч-175
TjaofV 2	- |	|	-	w1/5
Ini
So D decreases n4/5times and т] increase n ^times.
(a)	DaVVfaVpV7
Thus D remains constant in the process pV3 = constant
So polytropic index n « 3
(b)	T] a VT a VpV
262
2.240
2.241
2.242
So T] remains constant in the isothermal process pV- constant, n - 1, here
(c) Heat conductivity к « т] Cv and Cv is a constant for the ideal gas Thus	n « 1 here also,
1ч/ПГ m я 2 л/m kT 1 з ▼ лт <2 л J2' 3 V яз
or
/ 2	/	2	x 8‘31 x 273 x 10~3\
(з-qj ( л3 j ” (3 x 18-9 X J ( л’хЗбхЮ46 J
,	. 1/2 ,	.1/4
<n-to( 2	\	/4 x 83-1 x 273\
- 10	-——--	----5------ - 0-178 nm
(3x18-9 J ( л’х-36 )
1 .
К - у <V > Л P Су
1. /вТт 1 cv
- TV-------7=--тиТ7
3 v тл y2nd2n М
(	cv\
Cv is the specific heat capacity which is —I. Now Cv is the same for all monoatomic gases such as He and A. Thus
ка7^
or
— - 8-7 KA
Vm* d2 j— d2 dx. dH*
1-658 - 1-7
In this case
v _?—1
1 ?? '1'2
4лт]0)
жг 2RAR „	жг 2лпсоЯ3
or	N<-----т— - 4 л -n co or N< - ---------
1 R4 1	1 AR
To decrease Np n times T] must be decreased n times. Now т] does not depend on pressure
until the pressure is so low that the mean free path equals, say, AR. Then the mean free
path is fixed and T] decreases with pressure. The mean free path equals ~ AR when
1
z я d2nQ
AR (nQ « concentration)
263
Corresponding pressure is pQ «
yflkT nd2 &R
The sought pressure is n times less
V2kT	10"23	n_._
p- -----------= 70-7 x-----0-71 Pa
nd2nbR	10"18xl0‘3
The answer is qualitative and depends on the choice for the mean free path.
2.243 We neglect the moment of inertia of the gas in a shell. Then the moment of friction forces on a unit length of the cylinder must be a constant as a function of r.
So,	2яг n —- M or (o(r)« ----------
dr 1	4 лт] I r2l
.	/1 j\	Nr /1 i\
and	Ш-Т~-----	0Г Пя Й-----12--2
4ЯТ1^1 Г2)	4л<0^ r2J
2.244 We consider two adjoining layers. The angular velocity gradient is So the moment of
the frictional force is a
кт C j & лг\а4(й
r-lnrdr ^r---^-
0
2.245 In the ultrararefied gas we must determine t) by taking X.  у h. Then
1 , /SkT 1 , mp	1 -/2Л?"
t) = TV----- хТЛхT V^pt
3 ’ nrn	2 kT	3 ’ nRT
so,
1	4 л
Ы-ЗтарУ^Т
2.246
From the formula
Take an infinitesimal section of length dx and apply Poiseuilles equation to this. Then dV -ла4 dp dt * 8r| Эх pV.RT^ RT pdV^ ^dm M dm	ла4М pdp
dt “ И" ~St\RT dx
This equation implies that if the flow is isothermal then p must be a constant and so
or
I ~2 „2I •P2~.P1 equals ———L in magnitude.
Thus,
лаМ 1P2 ~
Ц° 16i]Pr 1
264
2.247 Let T« temperature of the interface.
Then heat flowing from left = heat flowing into right in equilibrium.
K2
T,-T T-T. I li	l2 ,
Thus, к,—-—» к, —r-2- or 7 = — -r—
Z1	Z2	fKl *2^
k + d
2.248 We have
Tr-T T-T2
Ki~/	“ K2~J	” K i +i
l2 ' Z1 + Z2
or using the previous result
Ki Л K2^2 ' h h
к
т\-т2
/i + /2
or
(7\ T2) T - T	I +1
K1 z2	21 y2	Z1 + Z2
---------------« K ------ or K- -J----------r-/1 K2 +l2	4 l2
h	K1 -K2
2.249 By definition the heat flux (per unit area) is ’ dT d	In ^1/^2
2 = -K — - - a — In T = constant = + a---------------
dx dx	I
Integrating
X ^2 InT- jlnyF + lnTj
where Tr  temperature at the end x « 0
, ,x/l
(ТЛ . alnTi/T,
T= Ti zr and 2=------------
Hi/	z
So
2.250
Suppose the chunks have temperatures Tlt T2 at time t and Tr - dTt, T2 + dT2 at time dt + t.
Then
C.dT^ C2dT2-^(Ti-TJdt
Thus

AT dt where AT = Tx - T2
Hence
AT- (A7)0e-</T where	+
265
2.251
А дТ л дТ
Q- к — - -АУТ —
Эх	Эх
2 л дт3/2 (Л	. м
« - —А —-—, (А « constant) 3 дх
2 , (Т3/2-Т3/2)
ж зА------1-----
Thus	Тзл - constant - у / Т3/2 - Т%/2
2.252
2.253
or using	Т = Tr at х = О
/	'		D 1
ly/SRT 1	2	R3/2iT3/2
3 лМ y/2nd2nmn М “ Зд3/2d2^MNA
Then from the previous problem
2iR3/2(T%/2-Tl/'2)
2/^ n ~	tж 3 here.
9n3/2d2VMN.l
(Л + t2)
At this pressure and average temparature « 27°C « 300K « T«-------------
1 kT	<
“7=—~— = 2330 x 10 “ 5m = 23-3mm > > 5-0mm = I y2 nd2 P
The gas is ultrathin and we write X = ~ / here
I
Then
dT
where
1	1 , MP R
к = — < V> x — lx —— x  X ,,
3	2 RT y-l M
P<v>_. I
6T(Y-1)
and
p<v>
6Г(у-1)
{Т2-Тг)
9
1
where
\/8RT	+
< v > « V -rj—. We have used T, - T. « —-—- here.
Мл	2	1	2
266
2.254 In equilibrium 2лгк — « -A« constant So T«	—Inr
ч	dr	2лк
But T = Tj when r « Rr and T* T2. when r »B2.
From this we find T«
2 dT
2.255 In equilibrium 4лг к — « - A « constant
2.256
T- B + -£-~ 4лк r
Using T - 1\ when r - Rr and T - T2 when r • jR2, T2 - T. / i i \
B2 Rr
The heat flux vector is - к grad T and its divergence equals w. Thus
V2T- --к
or	----r	— in cylindrical coordinates.
r dr I dr I к 7
or	T~B + Alnr-^-r2
2x
Since T is finite at r « 0, A * 0. Also T» To at r • R
so	B= То + -^-Я2
u 4k
Thus	T~ T0 + ^-(R2-r2)
u 4k
r here is the distance from the axis of wire (axial radius).
2.257 Here again
V2T-к
So in spherical polar coordinates, or Again so finally
1 JL z°L dr I Г dr
-—or к
2 Ol_ dr
W 3	.
- — r+A 3k
Т.1.* r OK
0 and B = Ta + ^-R2 u 6k
T~ Tn + ^-(R2-r2
PART THREE
ELECTRODYNAMICS
3.1 CONSTANT ELECTRIC FIELD IN VACUUM
3.1 Fd (for electoms) - —-—у and F =
4 л e0 r F r
Thus
Fd
—— (for electrons) -
Fgr
----------T
4 л e0 у m
(1-602 x 10"19 C)2
4 x 1042
—x 6.67 x 10 ’11 m3 / (kg • s2) x (9-11 x 10 " 31 kg)2
9 x 10 J	'
Similarly
F<1 „ t 4 q2
-=- (for proton) - --2--
*gr	4ne0ym
_____________________________________________________i x 10м (——T j x 6-67 x 10 11 m’/(kg  s2) x (1-672 x 10 " 27 kg)2 19 x 10 I
ForFd- Fr
в2 Y m2 q г-----------------
— —r- J-r- or =L» V4ne0y 4ЯС0Г2 r2 «	°
- V6 67x l---lm^kg~s2) . o-86 x 10 ’10 C/kg
9 x 109
1	23
3.2 Total number of atoms in the sphere of mass 1 gm « —x 6 023 x 10 63o4
23
So the total nuclear charge X » ——---x 1-6 x 10”19 x 29
Now the charge on the sphere ~ Total nuclear charge - Total electronic charge
268
3J
3.4
6-023 х 1023 t, _n_w 29x1	1n2_
—6У54~X145X 10	X ЛоГ" 4298 X 10 C
Hence force of interaction between these two spheres,
F - —— • [4 398*10 ]2N . 9 x IO9 x 104 x 19-348 N - 1-74 x 1013 N 4ле0 i2
Let the balls be deviated by an angle 0, from the vertical when separtion between them equals x
Applying Newton’s second law of motion for any one of the sphere, we get,
T cos 0 * mg
and	T sin 0 » Fe
From the Eqs. (1) and (2)
tanO
mg
But from the figure
tan 0 »
2
 — as x « 21
(1)
(2)
0)
/	(4)
From Eqs. (3) and (4)
or
2/ 4леох2 2/
Thus
/
Differentiating Eqn. (5) with respect to time dq In^mg 2q dt 'l " dt
dx According to the problem —
ч 1/2 dq 3xt0mg 2 a dt" I yfe
a/Vx (approach velocity is )
so,
„ dq 3 \l2m0mg
Hence, , » a V -------;----
9 dt 2 I
Let us choose coordinate axes as shown in the figure and fix three charges, qr, q2 and q3 having position vectors r2 and r3 respectively.
Now, for the equilibrium of q3 + ?2?3 (*?-»?) 91?з('Т-'Т)
I -* -*| 3	+ I -» -»|3	" u
^3 Ъ.-'тз #2
>
269
or,
because or, or,
*?2	41
Vfc (/Т-гГ)-
Also for the equilibrium of qp
43 (?) 4z (К- ?)
I'T-'Tl3 + I'^-'Tl3
or>	%>= .^%al^-?l2
lr2 - ri I
Substituting the value of r£, we get, ~4i42 93	+ Л2 )2
3.5 When the charge qQ is placed at the centre of the ring, the wire get stretched and the extra tension, produced in the wire, will balance the electric force due to the charge qQ. Let the tension produced in the wire, after placing the charge qQt be T. From Newton’s second law in projection form Fn » mwn.
TdQ--r~^
4 л e0 r2
(dm) 0,
or,
44o
8 л2 e0 r2
3.6 Sought field strength
Z= —------
43ieo|r-r0|2
« 4-5 kV/m on putting the values.
3.7 Let us fix the coordinate system by taking the point of intersection of the diagonals as the origin and let к be directed normally, emerging from the plane of figure.
Hence the sought field strength :
270
q li + xk -q l(- i)+xk
~ 4ле0 (/2+x2)3/2+ 4ne0 (Z2 + x2)3/2 —> —»	—>	—>
-q Ij + xk q l(-j )+х к + 4ле0 (Г+?)зл + 4ле0 (Z2 + x2)3/2
= 4 tr ₽ <72 4. v2\3/2	~
4 71 8q \l + X )
Thus E = -)------------5-475-
V2 ле0 (Z2 + x2)3/2
3.8 From the symmetry of the problem the sought field.
£ = f dEx
where the projection of field strength along
x - axis due to an elemental charge is
я/r _ cos ® _ ЯR cos &dQ
x 4 л e0 R 2	4 7t2 e0 R 3
Hence
E =
Я 4тс2 £0jR2
£ cos 0 dQ
л/2
4 2^z0R2
3.9 From the symmetry of the condition, it is clear that, the field along the normal will be zero
i.e.	En = 0 and £ = E,
Now	dE, = ----------z— cos 0
1 4ле0(Л2 + /2)
But	dq =	- - dx and cos 0 = —«—o
4	2^R	(R2±l2)1/2
271
dE
For £max, we should have = 0
So,(Z2+Л 2 )3/2 - | Z (Z2+ Л2 )1/2 2Z - 0 or Z2+/?2-3Z2-0
Thus Z = ~j= and E - —7—^-5
V2 m“ б73ле0Л2
3.10 The electric potential at a distance x from the given ring is given by, ф (x) = —--------------------------------------?---
4леох 4л80(Л2 + х2)1/2
Hence, the field strength along x-axis (which is the net field strength in 'our case), F = _^= q 1__________________________________qx_______
x dx 4леоХ2 4 л e0 (Л 2 + x2 )3/2
?(Я5 + ?)3/2
g Г 3 ^2 3 7?4	1
4 л е0 2 х2 8х4 "’J
Neglecting the higher power of R/x, as x » R.
E-^.
8 7t E0X
Note : Instead of ф (x), we may write E (x) directly using 3.9
3.11 From the solution of 3.9, the electric field strength due to ring at a point on its axis (say x-axis) at distance x from the centre of the ring is given by :
£(x)----------™
,	4ле0(Л2 + х)3
And from symmetry E at every point on the axis is directed along the x-axis (Fig.).
Let us consider an element (dx) on thread which carries the charge (\dx). The electric force experienced by the element in the field of ring.
dF = (Xdr)E(x)» -------
4 л e0 (A2 + x2)372
Thus the sought interaction 00
F_ I \qxdx
J 4 л e0 (Я 2 + x2)372
0
On integrating we get, F = —	—
272
3.12 (a) The given charge distribution is shown in Fig. The symmetry of this distribution —>
implies that vector E at the point О is directed to the right, and its magnitude is equal to —>	—>
the sum of the projection onto the direction of E of vectors dE from elementary charges —>	-—>
dq. The projection of vector dE onto vector E is
1 dq dE cos op = ------cos op,
4ле0т?2
where dq = kRdy= Xq R cos cp d <p.
Integrating (1) over ф between 0 and 2 л we find the magnitude of the vector E:
2 я
2 j ^0 cos ф d ф =  -----—.
о
It should be noted that this integral is evaluated in the most simple way if we take into account that <cos2 ф > = 1/2. Then 2я
cos2 ф d ф » <cos2 ф> 2 л « л.
0
£ = ---—
4 л ел R
(b) Take an element S at an azimuthal angle ф from the x-axis, the element subtending an angle d ф at the centre.
The elementary field at P due to the element is
Xq cos ф d ф R
4 л e0 (x2 + R 2 )
along SP with components
cos ф d ф R -------z----7- x { cos 0 along OP, sin 0 along OS } 4 л e0 (x2 + л )
.	л	x
where	cos 0 «	----5—гто
(х2 + Я2)1/2
2 л
The component along OP vanishes on integration as I cos ф d ф « 0
о
The component alon OS can be broken into the parts along OX and OY with R 2 cos ф d ф
--------z---x { cos ф along OX, sin ф along OY }
4 л e0 (x + R )
On integration, the part along OY vanishes.
Finally	/T\
E-E	Sr>rA-------------=-
Forx»« ’ ’	JJbJ 35
£ = —£—T where p = л R 2
4 л e0 x	Л
273
3.13 (a) It is clear from symmetry considerations that vector E must be directed as shown in the figure. This shows the way of solving this problem : we must find the component dEr of the field created by the element dl of the rod, having the charge dq and then integrate the result over all the elements of the rod. In this case
dEr - dEcosa- —^—cosa,
4*e0
wherek « is the linear charge density. Let us reduce this equation of the form convenient
for integration. Figure shows that dl cos a « r0 d a and
Consequently,
1 Xroda x	.
dE, = --------z— « ---------cos a a a
4 л e0 r2 4 л e0 r
This expression can be easily integrated :
E = -r—— 2 f cos a d a » ——— 2 sin an 4 л e0 r J	4 л e0 r u
о
where a0 is the maximum value of the angle a,
0 cos Ct
sina0- a / va2 + r2
™	с- q/2a „ a
Thus, E = ,----2 —
4яепг ,/T
- .1 ......
4леогУа2 + г2
Note that in this case also E ~	—7 f°r r»a as of the field of a point charge.
4 я e0 r
(b) Let, us consider the element of length dl at a distance I from the centre of the rod, as shown in the figure. Then field at P, due to this element. CZ kdl
4 Я Eq (r - l)^ k*
if the element lies on the side, shown in the diagram, and dE « ----------у, if it lies on
4 я e0 (r + Г)
dl ул t—l—> la
V
. dE
other side.
I Kdl
J J 4ле0(г- I)2 J 4яе0(г + /)2 о	0
On integrating and putting к « we get, E «	----2--2
2a	4 л e0 (r _ д )
Г	4
г» a, E « ------—y
4 я eor
Hence
£-
kdl
For
1
274
3.14
The problem is reduced to finding Ex and Ey viz. the projections of E in Fig, where it is assumed that к > 0.
Let us start with Ex. The contribution to Ex from the charge element of the segment dx is
1 Kdx .
dE = --------z-since
4 л e0 r2
(1)
Let us reduce this expression to the form convenient for integration. In our case, dx = r da/cos a, r = y/cosa. Then
dEv « -—-— sin a J a.
4л£0у
Integrating this expression over a between 0 and л/2, we find
Ex= к/4ле0у.
In order to find the projection Ey it is sufficient to recall that dEv differs from dEv у	x
in that sin a in (1) is simply replaced by cos a.
This gives
dEy = (kcos a da )/4 л e0у and E = k/4 л e0y.
We have obtained an interesting result :
Ex = Ey independently of y, —>	—>
i.e. E is oriented at the angle of 45° to the rod. The modulus of E is
E- ^/e2+E2 ~
x r у
kV2/ 4 л £oy.
3.15
(a)	Using the solution of 3.14, the net electric field strength at the point О due to straight parts of the thread equals zero. For the curved part (arc) let us derive a general expression i.e. let us calculate the field strength at the centre of arc of radius R and linear charge density X and which subtends angle 0O at the centre.
From the symmetry the sought field strength will be directed along the bisector of the angle 0O and is given by
+ V2
/k(AJ0)
—-----cos 0 =
4 л en R
-^2
к . 00
-------— sin —
2 л e0 R 2
In our problem 0O = rt/2, thus the field strength
due to the turned part at the point
V2 к 4 л eqR
о ~
which is also the sought result.
(b)	Using the solution of 3.14 (a), net field strength at О due to stright parts equals / -y/2 к \ к
V2 --------- = --------- and is directed vertically down. Now using the solution of 3.15
|4ле0Л1 2ле0Л	j	ь
275
(a), field strength due to the given curved part (semi-circle) at the point О becomes -------— and is directed vertically upward. Hence the sought net field strengh becomes 2 7t Eq Л zero.
3.16 Given charge distribution on the surface_o = a • r is shown in the figure. Symmetry of this distribution implies that the sought E at the centre О of the sphere is opposite to a dq = о (2 л r sin 0) r d 0 = (a • r*) 2 л r2 sin 0 d 0 = 2 л a r3 sin 0 cos*© d 0
Again frgin symmetry, field strength due to any ring element dE is also opposite to a i.e. dE f | a. Hence
_______ dq Г COS 0____________-5tT. л	г a
= --------; 2	—2-----Т^з/i	the result of 3.9)
4 я e0 (r sm 0 + r cos 0) a
_ (2 лаг3 sin 0 cos 0 df 0) r cos 0 (- a*) 4 л e0 r3	a
a r	2jn
-— sm 0 cos d 0
2 Eq
Thus
E - Г dE ( - Г Г sin 0 cos2 0 J 0
J 2e0 J о
т	7* ar 2 ar
Integrating, we get	—
ZEq J J£ 0
3.17
We start from two charged spherical balls each of radius R with equal and opposite charge densities + p and - p. The centre of the balls are at + and- respectively so the  R or r — ^cos0« R and r + ~cos0« R, considering
equation of their surfaces are r - j
a to be small. The distance between the two surfaces in the radial direction at angle 0 is
| acos0 | and does not depend on the azimuthal angle. It is seen from the diagram that the surface of the sphere has in effect a surface density a = o0 cos0 when
a0 = pa.
Inside any uniformly charged spherical ball, the field is radial and has the magnitude given by Gauss’s theorm
2г 4тг 3
4лг E - -j-r p/e0
or
Pr
3eo
In vector notation, using the fact the V must be measured from the centre of the ball, we
get, for the present case
AZ
276
3.18
Q° = -pa/3e0 = —к
When F*is the unit vector along the polar axis from which 0 is measured.
Let us consider an elemental spherical shell of thickness dr. Thus surface charge density of the shell о = p dr = (5*- r*) dr.
Thus using the solution of 3.16, field strength due to this sperical shell
л dE = - -— dr 3e0
Непсе the sought field strength
R
7? a* C , a*R2
E = --— I rJr« --—.
3 Cq	6 £q
0
3.19	From the solution of 3.14 field strength at a perpendicular distance r <R from its left end E(r) =	(-0 + 7-^— (er)
4 л e0 r 4 л e0 r r
Here er is a unit vector along radial direction.
Let us consider an elemental surface, dS - dydz* dz(rdG) a	figure. Thus
3.20	Let us consider an elemental surface area as shown in the figure. Then flux of the vector —>
E through the elemental area,
</ф= E-dS*= 2£0cos tpdS (as	f dS*)
----------5--5—1/7 (r 6) dr 4ле0(/2 + ?) (P + r2)172
2ql r dr dd 4 л e0 (r2 +12 )3/2
277
where EQ = -------\—z— is magnitude of
4 я e0 (/ + r )
field strength due to any point chaige at the point of location of considered elemental area.
R	2л
Thus Ф =
rdr
(r2 + /2)3/2
2д/х2я
4 я e0
r dr _ <7 (r2 + Z2)3/2“ £0
I y/l2+R2
It can also be solved by considering a ring element or by using solid angle.
3.21 Let us consider a ring element of radius x and thickness dx, as shown in the figure. Now,
flux over the considered element, d® = ~E-dS = ErdS cosG
But E„ = from Gauss’s theorem, r 3e0
ro
and dS = 2 я x dx , cos 0 = — r
r™	pr ~	, ro
Thus аФ = zr— 2 я x dx — = 3e0 r
Hence sought flux
2лрг0 f Ф = —----- I x dx
3e0 J о
2 я p z*o (Д “ ^0 )
3 e0 2
-—2 nxdx 3e0
3.22 The field at P due to the threads at A and В are both of magnitude
_________к__________
2л£0(? + /2/4)1/2
and directed along AP and BP. The resultant is along OP with
278
3.23
Take a section of the cylinder perpendicular to its axis through the point where the electric field is to be calculated. (All points on the axis are equivalent.) Consider an element 5 with azimuthal angle qp. The length of the element js K/7qp ,R being the radius of cross section of the cylinder. The element itself is a section of an infinite strip. The electric field at О due to this strip is 0q cos qp (R Jqp) --------------------- along SO 2ntQR &
This can be resolved into
ao cos qp J qp (со8ф along OX towards О sinqp along YO
2 Л Eq
On integration the component along YO vanishes. What remains is
3.24
3.25
2л
I a0 cos2 qp Jqp
J 2 л Eq о
ao
= -— along XO i.e. along the direction qp = л.
2 Eq
Since the field is axisymmetric (as the field we conclude that the flux through the sphere of radius R is equal to the flux through the lateral surface of a cylinder having the same radius and the height 2K, as arranged in the figure.
Now,
But
t'-ii
-S~ ~2xR-2R-4xaR R R
(a) Let us consider a sphere of radius r <R then chaige, inclosed by the considered sphere,
Thus
= J 4 3tr2drp = J 0	0
Now, applying Gauss’ theorem,
Er4itr2 =	, (where Er is the projection of electric field along the radial line.)
r	eo
dr
(1)
or,
— f 4лг2(1 dr I KI
о 4	'
Po
3 £0
4R>
279
And for a point, outside the sphere r>R. R
I 2 l Г 1
i ed = J & r Po 1 ~ (as ^ere *s no с^аг8е outside the ball) о	'	'
Again from Gauss’ theorem, R
Er 4 яг2
0
_ Po [«'
£r= — ' r eoL
Po^
12 r2 e0
(b) As‘magnitude of electric field decreases with increasing r for r>R, field will be maximum for r<R. Now, for Er to be maximum, d (	3r2\	3r
*ëà “ '-M-“ “
E -max q c у e0
or,
Hence
3 4R
2R 3
3.26 Let the charge carried by the sphere be q, then using Gauss’ theorem for a spherical surface having radius r > R, we can write.
г-, л 2	<1 inclosed	q
Е4лг = -----------=
eo	eo
1 I а .	2 ,
— I — 4л r dr
r R
On integrating we get, „ .	2 (q - 2 л a R2)
Е4лг = —--------------
4 лаг2
Eq	2 £q
The intensity E does not depend on r when the experession in the parentheses is equal to zero. Hence
q = 2 л a R2 and E =
2e0
3.27 Let us consider a spherical layer of radius r and thickness dr, having its centre coinciding with the centre of the system. Then using Gauss’ theorem for this surface,
Г» A 2 qinclosed	I О d V
Е,4лг = -----------= I r------
e0 J e0
0
— Г poe 4 л r2 dr
Eo J
0
280
After integration
/7 A _ 2 P 4	a r3
•J Cq la
it ^0 н - a / i or, £r=-----------[1-e	]
3 ea r
Now when a r3 < < 1, £ « r . 3 e0
And wnen a r3 > > 1, Er~ ---——
3 Eq cl r
3.28
3.29
Using Gauss theorem we can easily show that the electric field strength within a uniformly charged sphere is E « r*
13 e0 j
The cavity, in our problem, may be considered as the superposition of two balls, one with the charge density p and the other with - p.
Let P be a point inside the cavity such that its position vector with respect to the centre of cavity be r_ and with respect to the centre of the ball r\. Then from the principle of superposition, field inside the cavity, at an arbitrary point P,
—>
Note : Obtained expression for E shows that it is valid regardless of the ratio between the radii of the sphere and the distance between their centres.
Let us consider a cylinderical Gaussian surface of radius r and height h inside an infinitely long charged cylinder with charge density p. Now from Gauss theorem :
E 2xrh = eo
(where Er is the field inside the cylinder at a distance r from its axis.)
„ ~ t. рлг2Л „ pr or, E 2 я rh = ------ or Er = 77—
e0	2 e0
Now, using the method of 3.28 field at a point Д inside the cavity, is
281
3.30 The arrangement of the rings are as shown in the figure. Now, potential at the point 1, qpx« potential at 1 due to the ring 1 + potential at 1 due to the ring 2.
.........+_________z?_________ 4ле0Я 4 л e0 (К2 + a2)172
Similarly, the potential at point 2,
- q ______________q__________
ф2 " 4 л e0 R + 4 л e0 (K2 + a2)172
Hence, the sought potential difference, qpi - qp7 = Аф = 2 I -—~	—7777 I
|4л£0/? 4л£0(Я2 + а2)1/2]
- ..g... fl-—1
2 л R 4	“ T
0 Vl + (a/R)2
\
3.31
We know from Gauss theorem that the electric field due to an infinietly long straight wire, at a perpendicular distance r from it equals, Er « 7—^—. So, the work done is Л Л £q T
2	n*
(where x is perpendicular distance from the thread by which point 1 is removed from it.)
Hence
л	X 1
д Ф12 = 3V711,11 «IV Cq
3.32 Let us consider a ring element as shown in the figure. Then the charge, carried by the element, dq = (2 л R sin 0) R d 0 0, Hence, the potential due to the considered element at the centre of the hemisphere,
,	1 dq 2jtnJ?sm0d0 uR . Q
t/фж -----^-ж ----------« -—sin0J0
4 л e0 R 4 л £0	2 £0
So potential due to the whole hemisphere
я/2
Яа f . Q <JR
<ржтг1 s,n 0 d 6  7Г
z, tQ	Z t0
0
Now from the symmetry of the problem, net electric field of the hemisphere is directed towards the negative у-axis. We have
1 d q cos Q or . Q л jn dEv = ---------— = -— sm 0 cos 0 dQ
y 4 Л so R2 2 Eo
л/2	x/2
Thus E = EJ = I sin 0 cos 0 d 0 = I sin 2 0 d 0 =	, along YO
y 2e0 J	4 e0 J	4 £0
о	0
282
3.33
3.34
Let us consider an elementary ring of thickness dy and radius у as shown in the figure. Then potential at a point P, at distance I from the centre of the disc, is
. o2nydy 4ле0(у1 2 + /2)1/2
Hence potential due to the whole disc, R
2
I a 2 nydy al Ф J 4ле0(у2 + /2)1/2 2e0
From symmetry
when /—*
a A2
a A2 _ Фя ~л-->>
4е0/
By definition, the potential in the case of a surface charge distribution is defined by integral
(p - —— I In order to simplify integration, we shall choose the area element dS 4 7t 8g J Г
in the form of a part of the ring of radius r and width dr in (Fig.). Then dS • 20 r dr, r = 2R cos 0 and dr « - 2R sin 0 d 0. After substituting these expressions into integral
1 i cr dS _ . ,	• r * *1.	• zn
qp « ------- I ------, we obtain the expression for ф at the point O:
4 71 Eq Г 0
Ф = - I 0 sin 0 d 0.
Л Eg J
Я/2
We integrate by parts, denoting 0 « и and sin 0 d 0 - dv :
J*0 sin 0 J 0 - - 0 cos 0
+J* cos 0 d 0 ж - 0 cos 0 + sin 0
which gives -1 after substituting the limits of
integration. As a result, we obtain
ф = aR/я e0.
283
3.35 In accordance with the problem qp * a • r
Thus from the equation : E « - V qp
E= - -^(axx)i + — (ayy)j + — (aIz)k
-[axi + ayj + 2k ]= -a
3.36 (a) Given, qp - a (x2 - y2)
So,	E = - Vqp * - 2 a (x i - у j )
The sought shape of field lines is as shown in the figure (a) of answersheet assuming a > 0:
(b) Since qp « oxy
So,	E = - V qp * - ay i - ax j
Plot as shown in the figure (b) of answersheet
3.37 Given, qp = a (x2 + y2) + bz2
So,	E « - Vqp = - [2 ax i + 2 ay j + 2 bz к ]
Hence	|E| - 2 V«2z2
Shape of the equipotential surface :
Put	p*« xi+yj or p2 - x2 + y2
Then the equipotential surface has the equation
a p2 + b z2 - constant - qp
If a > 0, b > 0 then qp > 0 and the equation of the equipotential surface is
p z2 qp/д + qp/b
which is an ellipse in p, z coordinates. In three dimensions the surface is an ellipsoid of revolution with semi- axis ^Jy/a , Vqp/fl , Vqp/b.
If a > 0, b < 0 then qp can be 0. If qp > 0 then the equation is
JL.-ZL.i qp/д qp/|b|
This is a single cavity hyperboloid of revolution about z axis. If qp = 0 then др2 - |b| z2 « 0
or	z-	P
is the equation of a right circular cone.
If qp < 0 then the equation can be written as
|b| z2 - a p2 - |<p|
z2 P2 ,
or	M Ф1 l<p| /«"
This is a two cavity hyperboloid of revolution about z-axis.
284
3.38
From Gauss’ theorem intensity at a point, inside the sphere at a distance r from the centre
is given by, Er» and outside it, is given by Er»	— —
3 Eq	' 4 Я Eq f
Potential at the centre of the sphere, ~	R	oo
(a)
Фо = J
0
E-dr
J 3e0 о
2*
I q J p R q
I  —n dr =	— + -—3——
J 4neor2 3e 2 4ле0Я R
as
(b)
q q iq	(
= —3-----+ —3-----» ----3—	as
8яе0Я 4ле0А	8яе07? I
Now, potential at any point, inside the sphere, r	<*>
at a
3 g )
4лЯ J
distance r from it s centre.
'0
dr
«о r2
3.39
On integration : cp (r) = 	3.
о Я ^qR
2 1
1--Ч
3R2
Фо 1-37?2
Let two charges +q and -q be separated by a distance I. Then electric potential at a point at distance r > > I from this dipole,
ф(г)«—+ —^----3-4 Я Eq Г+ 4 Я Eq r_ 4 Я Eq
But
I cos 0 and r+ r_ «
From Eqs. (1) and (2),
—> —> , 4 q I cos G p cos G p • r ф (r) = -----у = -----t<P “ —----
4яе0г 4ле0г 4яб0г
where p is magnitude of electric moment vector. Эф _ 2 p cos G &r 4 я e0 r3
Now,
and
dtp _ p sin G r Э G 4 я e0 r3
(1)
-4,
Ф w

Eq “
P
2

E =
So
3.40
4-£q = ——3 v4cos2G + sin2G 4 Я Eq Г
From the results, obtained in the previous problem, „	2 p cos G j „ p sin G
E = ---------anc* = -------------г
4 Я Eq Г	4 Я Eq Г
From the given figure, it is clear that,
Ez = E cos G - Eq sin G = ——r (3 cos2 G - 1) 4 Я Eq Г
285
.	„ г пг Q	з р sin G cos G
and	= Er sm G + EQ cos G = —------=—
4 Л Eq Г
When	£1 p*, |E | = £± and Ez = 0
2	1
So	3 cos G = 1 and cos 6 = "7=
V3 - >
Thus ELp at the points located on the lateral surface of the cone, having its axis, coinciding with the direction of z-axis and semi vertex angle G = cos ’11 / V3~.
3.41 Let us assume that the dipole is at the centre of the one equipotential surface which is spherical (Fig.). On an equipotential surface the net electric field strength along the tangent of it becomes zero. Thus
г • n г л	г • n psinQ
- Eq sin G 4- Eq = 0 or - Ео sin G 4- -----7 = 0
4 л Eq r
Hence
Alternate : Potential at the point, near the dipole is given by,
1/3
Thus
___£__ 4ne0£0
286
Also,	<p» In I r +/72| - т~~In I r-Z?21
2яе0 1	1 2яе0 1 J
X , г2 + г Z cos 0 + ?/4 X Z cos 0	,
»  ----In-3------------=—- —--------, r»l
4ле0 /-r/cos 0 + /V4 2яеог
3.43	The potential can be calculated by superposition. Choose the plane of the upper ring as x ж 1/2 and that of the lower ring as x = - //2.
1ЪеП <₽” 4яе0 (Я2 + (x - 1/2)2 ]W ’ 4я e0 [J?1 + (x + Z/2)2 ]*
„	?_______,____________Я_____
4 л e0 [Я2 + x2 - Zr]1/2 4 л e0 [Я2 + x2 + lx]172
„ _______Я Л . lx \ _ q / ______________________________Zx___\
4лe0(Я2 + x2)1/2 (	2(R2 + x2)) 4ле0(Я2+х2)1/Д	2(R2 + x2) )
_ Ч &
" 4ле0(Я2+х2)М
For	Ix|»R, <p —
1	4ЯХ2
The electric field is E « -
ax
9Z	3 ql	ql(2x2-R2)
" 4 л e0 (RJ + xJ)M 2 (A2 + ?)S/2 4 я e0 4 я e0 (Я2 + x2)5/2
For	| x| »R, E— ———r.	The plot is as given in the book.
2 Л 80X
3.44	The field of a pair of oppositely chaiged sheets with holes can by superposition be reduced to that of a pair of uniform opposite charged sheets and discs with opposite charges. Now the charged sheets do not contribute any field outside them. Thus using the result of the previous problem R
I (-a)/2nrdrx Ч'} 4 л e0 (r2+x2)3/2 0 „2	2 R + x oxi Г dy	oxi *	4f /	x,3/2		 4e”< y	2SoV?t/ e .	.-giL i-		 x	дх	2e0 ул2 + х2 (^ч-х2)^2 The plot is as shown in the answersheet.	2e0(A2 + x2)3/2’
287
3.45	For x > 0 we can use the result as given above and write al /.	|x| \
<₽“± 2eo(1-(J?2+x2)l/2j for the solution that vanishes at a. There is a discontinuity in potential for | x| • 0. The solution for negative x is obtained by a -* - o. Thus
olx Ф = ----------z-777 + constant
2e0(£+x2)1/2
Hence ignoring the jump р dtp alR2 3x “ 2e0(£2 + x2)3/2
for large |x| <p*± ——z and E- --------—7 (where p = JtR2ol)
4яе0х	2ле0|х|
3.46	Here £ -	, £ft- £ = 0 and £-p^
r 2ле0г 0 v	r dl
(a) /Talong the thread. E does not change as the point of observation is moved along the thread.
F- о (b) p along r,
F- F --^P 	---^P-7 (on using ~-e*» 0
2ле0Г 2ле0Г \	Эг
(c) p*along 7* д к F = р----------------------------------е
гд62ле0г г =	Р^-Г-	Р^ .
2 л е^г2 а 0	2 л е0 г2 0	2 л е0 г2
3.47	Force on a dipole of moment p is given by, x-F = *11
In our problem, field, due to a dipole at a distance /, where a dipole is placed,
Hence, the force of interaction, F- -3p................................7~ 2-lxlO-16N
2 л e0Z
3.48	- d ф * E • dr^ a(ydx+xdy)~ ad (xy) On integrating,	Ф = - a xy + C
3.49	_ d ф ш £.	[2 a xy i + 2 (x2 - y2) j ] • [dx i' + dy j ]
or,	(/ф« 2axydx + a (x2-^2) dy • adtfy) - ay2 dy
288
On integrating, we get, <P =	+ C
3.50	Given, again —► __________________________—> —> —» —> —► —>
- d <p - E-dr* (ayi + (ax + bz) j + by k) • (dx i + dy j + dx k) « a (y dx + ax dy) + b(zdy + у dz) = ad (x y) + bd (yz)
On integrating, Ф = - (a xy + b yz) 4- C
3.51	Field intensity along x-axis.
£ = -^B-3ax2	(1)
x dx	v ’
Then using Gauss’s theorem in differential from p (x) —— = r so, p (x) = 6a eox. dx Cq
3.52	In the space between the plates we have the Poisson equation
Э2 ф _ Po ax2 ~ eo
Po 2 л П
or,	Ф= -----X 4- Ax 4- В
2e0
where p0 is the constant space charge density between the plates.
We can choose	ф (0) = 0 so В = 0
л xj Po^2 л Аф Po^
<p(d)= t^Ad-^- or, A=—+ —
£=	—x-A- 0 for x- 0
dx 80
Then Now if then
Also
2e0 Д ф Po T~
E(d)= Eo
3.53 Field intensity is along radial line and is Er--^- -2»r ' Sr
From the Gauss’ theorem, л 2 c Г dq 4 л r E- I r J eo, where dq is the charge contained between the sphere of radii r and r 4- dr. r
Hence 4 л r2 Er = 4 л r2 x (- 2ar) = — J r'2 p (r') dr’ 8° 0
(1)
Differentiating (2) p = - 6 e0 a
(2)
289
3.2	CONDUCTORS AND DIELECTRICS IN AN ELECTRIC FIELD
3.54	When the ball is charged, for the equilibrium of ball, electric force on it must counter balance the excess spring force, exerted, on the ball due to the extension in the spring.
Thus Fd = Fspr
2
or,----“----г = к x, (The force on the charge
4л6о(2/)2
q might be considered as arised from attraction by the electrical image) or,q = 4/Vji еокх, sought charge on the sphere.
gill!
I
ima§&
I
3.55	By definition, the work of this force done upon an elementry displacement dx (Fig.) is given by
dA = Fxdx =
4 л e0 (2x)
where the expression for the force is obtained with the help of the image method. Integrating this equation over x between / and oo, we find
q2 Г (jr q2
16 я e0 J x2 16 я £0 I *
3.56
(a) Using the concept of electrical image, iit is clear that the magnitude of the force acting
on each charge,
2	г
|F|= VI —2—2-----------
4яе0/ 4яе0 (
3.57
2
-----2—z(2V2-1) 8яе0/2
(b) Also, from the figure, magnitude of electrical field strength at P
E~	^“2
I 5 V5 I я e0 I
Using the concept of electrical image, it is easily seen that the force on the charge q is, _______________________________
4 л e0 (2/)1 4 я e0 (2 VI I)2 (2^2-l)q2 „ .
- ---------7— (It is attractive)
32яе0Г
-<—lk—> Fq S'
I	I
777^777777777777^77"
-----L----->1^
290
3.58
Using the concept of electrical image, force on the dipole p, —> dE
F » p , where E is field at the location of p*due to (-p*) dE dl
or, |F |
3p2
Р»-----e—
32 Л Eq Z
339
4k€0(2Z)3
To find the surface charge density, we must know the electric field at the point P (Fig.) which is at a distance r from the point О .
Using the image mirror method, the field at P9
E~ lEcvsa* 2---------—Х-» ----------
4ле0х2*	2 л e0 (Z2 + r2)3/2
Now from Gauss’ theorem the surface charge density on conductor is connected with the electric field near its surface (in vaccum) through the relation о - e0 where En is the projection of E onto the outward normal n^with respect to the conductor).
—>
As our field strength E f j n, so qi 2n(l2 + ri)i/2
(a) The force Fr on unit length of the thread is given by Fr - к Ег
where Ex is the field at the thread due to image charge :

3.60
a- - t0E

Thus
i
-X 2ne0 (2/) - k2 4ле0/
minus singn means that the force is one of attraction.
(b) There is an image thread with charge density- X behind the conducting plane. We calculate the electric field on the conductor. It is
£(х)-£я(х)----------3-
Jteo(jr + Z ) on considering the thread and its image.
Thus
, л	XZ
0(x)= e0£„=	2	2
n(x +1 )
291
3.61 (a) At О,
ЕЛ<Р)
X.
2яе0/
So
к Г xdx
2n:e0J (x2 + r2)372 I
Непсе
3.62 It can be easily seen that in accordance with the image method, a charge -q must be located on a similar ring but on the other side of the conducting plane. (Fig.) at the same perpendicular distance. From the solution of 3.9 net electric field at (9,
E « 2------—х-ттг (- **) where S*is
4ле0(Я2 + /2)372
outward normal with respect to the conducting plane.
Now	£ - —
* *o
He"“ ° -
where minus sign indicates that the induced carge is opposite in sign to that of chatge q> 0.
3.63 Potential ф is the same for all the points of the sphere. Thus we calculate its value at the centre О of the sphere. Thus we can calculate its value at the centre О of the sphere, because only for this point, it can be calculated in the most simple way.
1 q ф-^/+<р
(1)
292
where the first term is the potential of the charge q, while the second is the potential due to the charges induced on the surface of the sphere. But since all induced charges are at the same distance equal to the radius of the circle from the point C and the total induced charge is equal to zero, <p'« 0, as well. Thus equation (1) is reduced to the form,
1 1 cp =--------,
4 л e0 I
As the sphere has conducting layers, charge -q is induced on the inner surface of the sphere q and consequently charge + q is induced on the outer layer as the sphere as a whole is uncharged.
Hence, the potential at О is given by,
Ф = q I (~g) I q
vo 4яеог 4neojR1 4яе07?2
It should be noticed that the potential can be found in such a simple way only at O, since all the induced charges are at the same distance from this point, and their distribution, (which is unknown to us), does not play any role.
Potential at the inside sphere,
s h 42
4 я e0 a 4 я £0 b
Obviously	<pe= 0 for q2 =
When	r a b,
41
42
4i / b>
Ф = --------+---------= -------и - —
r 4 я e0 r 4 я e0 r 4 я e0 I a
r, using Eq. (1).
And when r z b
4i 42 4i /1 1 qp  ------j.-----= -----I — _ _
r 4яе0г 4яе0Ь 4яе01г a
3.66 (a) As the metallic plates 1 and 4 are isolated and conncted by means of a conductor, qpx = <p4. Plates 2 and 3 have the same amount of positive and negative charges and due to induction, plates 1 and 4 are respectively negatively and positively charged and in addition to it all the four plates are located a small but at equal distance d relative to each
293
other, the magnitude of electric field strength between 1-2 and 3-4 are both equal in
—>	—->
magnitude and direction (say E ). Let E’ be the field strength between the plates 2 and 3,
——►	—>
which is directed form 2 to 3. Hence E' f | E (Fig.).
According to the problem
E’ d= Дф « qp2 “ Фз	(1)
In addition to
<p1 _ <p4 - 0 - (<Pj - <p2) + (<p2 - <p3) + (<p3 - qp4) or,	0 = - Ed 4- Дф - Ed
or,	Дф = 2Ed or £ =
Hence	2d	(2)
(b) Since E a a, we can state that according to equation (2) for part (a) the charge on the рЦ1е 2 is divided into two parts; such that 1 / 3 rd of it lies on the upper side and 2 / 3 rd on its lower face.
Thus charge density of upper face of plate 2 or of plate 1 or plate 4 and lower face of
Eq Дф 2d
3a = e0 E =
and charge density of lower face of 2 or upper face of 3
a<_ e E>. e Asel ° eo£ 80 j
Hence the net chaige density of plate 2 or 3 becomes a + a' ®
3 e0 Д ф
which is obvious
from the argument
3.67 The problem of point charge between two conducting planes is more easily tackled (if we want only the total charge induced on the planes) if we replace the point charge by a uniformly charged plane sheet
Let a be the charge density on this sheet and Er, E2 outward electric field on the two sides of this sheet
Then E.+E?= — 1	2 £o
The conducting planes will be assumed to be grounded. Then Er x = E2 (I - x).
Hence	£i = 7^- (/ -x), E2 = ~-x
1	/е0	/e0
This means that the induced charge density on the plane conductors are
a1= -y(Z-x), o2= --x
Hence qY = - (Z - x), q2 “ - x
294
3.68	Near the conductor E ~ Е» — eo
This field can be written as the sum of two parts Er and E2. Er is the electric field due to an infinitesimal area dS.
Very near it * ± -—
2 Eq
The remaining part contributes E2 «
о
-— on
2 Eq
both sides. In calculating the force-on the element dS wc drop (because it is a self-force.) Thus
dF a o2
dS 2 Eq 2 Eq
3.69	The total force on the hemisphere is
jt/2
/	2
---cos G-27t£sinGAJG 2e0
о
x/2
2 я А2 о2 Г
2 Eq j
0
cos G sin G d G
2лЛ2 1 ( q \ q2 _____x__x I —*— I » • ..л.
2e0	2 (4ЛЯ2)	32ле0Я‘
3.70	We know that the force acting on the area element dS of a conductor is, cLF-^oEdS	(1)
It follows from symmetry considerations that the resultant force F is directed along the z-axis, and hence it can be represented as the sum (integral) of the projection of elementary forces (1) onto the z-axis : JF>JFcosO	(2)
For simplicity let us consider an element area dS • 2 л £ sin G Rd G(Fig.). Now considering that E « o/Eq. Equation (2) takes the from 7Г rr2 R2 dF~ ——— sin G cos G d G eo
/ я Oq R2 ' \ e° >
cos3 G d cos G
296
Integrating this expression over the half sphere (i.e. with respect to cos 0 between 1 and 0),
we obtain
F-Fz-
Л Uq/?2 4e0
3.71
nop
The total polarization is P = (e - 1) eqE. This must equals	where nois the concemtation
of water molecules. Thus

(e-1)e0£
- 2-93 x
103 on putting the values
3.72	From the general formula
4ле0 /
7*	1	-»
E - -------v, where r * I and r 11 p
4ле0 z3’
This will cause the induction of a dipole moment. -	„ i 2p*
Thus the force, _L1&A_JL_2&= зр/ 4 л i3 dl 4 л e0 i3 4л2е0/7
3.73	The electric field E at distance x from the centre of the ring is,
E to----------j/i
4ле0(Я2 + х2) 2
The induced dipole moment is p » В tnE » -a , „
°	4л(Я2 + х2)3/2
The force on this molecule is
F» — Е» ?₽*	? д x ш g2 P x (R2 - 2x2)
‘Рдх ‘ 4л(Я2+х2)’44я£оах(Я2 + х2"/2' 16л2e0 (f?2 + x2)4
± R
This vanishes for x « (apart from x « 0, x « oo)
It is maximum when a x(r2-J*2) to (Я2 + х2)4
or, (R2-2x2)(R2 +x2)-4x2(R2 + х2)-8х2(Я2-2x2)« 0
n2	____
or,	A4-13x2/?2 +10x4= 0 or, x2= ^-(13± V129 )
Ot^ =	Vi3 ± V129 (on either side), Plot of Fx (x) is as shown in the answersheet.
296
3.74	Inside the ball
Also е0£ + Р=й or P= — D= —-Ц u	e	e 4 л r5
Also, q'= -ф p’-dT^ -	f dQ = -^~^q
J	e 4<rc J	e
3.75	^did “ ® ^O^diel ж & conductor ж ОГ> ^diel ж f F E t0
£ — I
P„- (e-l)^- ^-0
o' - - P  - ------ 0
n e
This is the surface density of bound charges.
3.76	From the solution of the previous problem q'^ « charge on the interior surface of the conductor
- - (e - l)/e fadS- - ^-q J	e
Since the dielectric as a whole is neutral there must be a total charge equal to
E — 1
q'„ = +-------Q on the outer surface of the dielectric.
л outer £ л
3.77
(a)	Positive extraneous charge is distributed uniformly over the internal surface layer. Let g0 be the surface density of the charge.
Clearly,	E = 0, for r < a
For a < r
e0 £ x 4 л r2 « 4 тс я2 o0 by Gauss theorem.
r 0 (a}	к
or, E = ------ “ , a<r<b
eoe
For r > b, similarly F °op\2	,
£» — - , r>b eo И
Now,
Or*
So by integration from infinity where ф (oo) « 0,
O»»1 h
Ф  -----r > b
eor
297
a0a2
-----+ B, В is a constant e e r
2 ,	2
or by continuity, <p = ------- — - — + ——
€q € I Г bl Eq b
For
A « Constant
2 , v 2
By continuity, ф  -------- — - — + ——
eoe la bl tQb
(b) Positive extraneous charge is distributed uniformly over the internal volume of the dielectric
Let p0 - Volume density of the charge in the dielectric, for a < r < b.
£ « 0, r < a
e0 e 4 л pE «	(r3 - a3) p0, ( a < r < b)
3
or,
3 e0 e
or,
E -	(b3 - a3) p0 / e0 4 я r2, r > b
(b3 - a3) p0
E « -------z— for r > b
3e0r2
By integration,
(b3-a3)Po
3t0r f°rr>b
or,
„ Po (r2 “ ф « В “ --- --+ --
T 3e0el2 r
By continuity
b3-a3	Po (b2
3 e0 b Po = " 3 e0 e I 2 + b
or,
B-
3eoe
e (b3 - a3) . (b2
2 + b
b
Finally
(a	\	2
a	21	n	Poa
— + a == В - -------, r < a
2	I	2 e0 e
On the basis of obtained expressions E (r) and (<p) (r) can be plotted as shown in the answer-sheet.
298
3.78	Let the field in the dielectric be E making an angle a with n. Then we have the boundary conditions,
Eq cos a0 = e E cos a and Eo sin c0 = £ sin a
So E = Eq V sin2 a0 + cos2 a0 and tan a - 8 tan a0 e
In the dielectric the normal component of the induction vector is
£>л= еое£л = e0e£cosa = e0E0cosa0
°' = Pn = Dn - eo£« = |1 - 7] e0£0 COS ao
8 — 1
3.79	From the previous problem,a' = e0------Eq cos 0
(a)	Then E’-dS=—Q^nR2E0cosQ^-
(b)	Ф D • dl* (Dlt -	) /a (e0 £0 sin 0 - e e0£0 sin 0) = - (e - 1) 80 £0 I sin 0
3.80	(a) divZ) = —- = p and D = p / dx
Ex =	, I <d and Ex = constant for l>d
e 8q	e0
Ф (x)« - £ -- , I <d and ф (x)  A -	, l>d then ф (x) -	(d-^--l\
2e eo	eo	eo I 2e I
by continuity.
On the basis of obtained expressions Ex (x) and ф (x) can be plotted as shown in the figure of answersheet.
299
(b)	p' = - div P = - div (e -1) e0 E • - p ~
o' - Pln -P2n, where n is the normal from 1 to 2.
« Pln, (P2 я 0 as 2 is vacuum.)
= (pd - p d/e) = ,p d e ~
—* I d 7
3.81 divP= D = p /dr r
r2Dr = p y+A Dr = | pr + ^, r<R
A = 0 as D. # oo at r • 0 .Thus, E,« —£— r	’	’ '	3 e e0
For	r>R, D -
/
By continuity of Dr at r = R ; B =
so,	Er =	0 , r>R
3e0?
n3
(px zP-, r>R and Ф»	+ C,r<R
3tQr	6 8 e0
R^“
C « + —L +	, by continuity of cp.
3 Eq 0 6 Eq
See answer sheet for graphs of E (r) and ф (r)
1 d \r3 L 1\1 p (e -1)
(b) p-dtvP---, —	-L
3.82	Because there is a discontinuity in polarization at the boundary of the dielectric disc, a bound surface charge appears, which is the source of the electric Held inside and outside the disc.
We have for the electric field at the origin.
where r - radius vector to the origin from the element dS.
300
a' » Pn - P cos 0 on the curved surface (Pn = 0 on the flat surface.)
—1►
Here 0  angle between r and P
By symmetry, E will be parallel to P. Thus 2x
/Р cos 01? tZ 0-cos 0 j ----------------9-----d
4 ле0Р о
where, r~R if d«R.
So,
Pd л 7* Pd En -------and E ж —-----
4e0E	4e0E
3.83	. Since there are no free extraneous charges anywhere
dDx
divD- ——== 0 or, D « Constant dx	x
But
Thus,
So,
Hence,
Dx - 0 at oo, so, Dx - 0, every where.
<P-
eo -3eod2
+ constant
3.84	(a) We have Dx * D2, or, e E2 я
Also,	E1^^E2~^ EQd or, E1+E2-2E0
2En	2eE0	2ee0E0
Hence, E2-----7 and E.-------- and D. - D2 - -
2	e+1	1	e+1	1	2	e+1
(b) Dy « D2, or, eE2« Ej« —  Ео eo
^0
Thus,	Ex  Eq , E2 - — and	« D2 * e0E0
301
3.85 (a) Constant voltage acros the plates; ж ^2 ж ж Бо^о> ж ео 8 ^о-
(b) Constant charge across the plates;
ж	ж e0^P &2 ж e е0^2 " C
Er (1 + e) - 2 £0 or EY - E2 -
3.86
3.87
At the interface of the dielectric and vacuum, ^1/" &2t The electric field must be radial and
Er • E2 *	.о > a<r<^
eoer
Now, q~ -^(2яЯ2) + ^Ц(2яЛ2)
« a(i +-hn
I e J
or, E1  £7 = -----?-----
2 л e0 r2 (1 + e)
In air the forces are as shown. In X-oil, (pn\
1 - —I
Since the inclinations do not change
1- 1-^ e “ p
Po < 1	e-1
or,	— - 1 - — « ---
p ее
e
or’ р-р°7^Т
where p0 is the density of X-oil and p that of the material of which the balls are made.
3.88	Within the ball the electric field can be resolved into normal and tangential components.
En • E cos 0, Et» £ sin 0
Then, Dn  e e0 £ cos 0
and Pn » (e - 1) e0£ cos 0
or, a' * (e - 1) e0£ cos 0
S0>	°m« “ (£ ~ О £0E>
and total charge of one sign,
302
1
q’ « $ (e - 1) e0E cos 0 2 JtR2 d (cos 0)« л R2 e0 (e - 1) E о
(Since we are interested in the total chaige of one sign we must intergrate co$ 0 from 0 to 1 only).
3.89	The chaige is at A in the medium 1 and has an image point at A’ in the medium 2. The electric field in the medium 1 is due to the actual charge q at A and the image charge q' at A'. The electric field in 2 is due to a corrected charge at A. Thus on the boundary between 1 and 2,
e?"- q-4 4'- q + q'
(a)	The surface density of the bound charge on the surface of the dielectric a s ^2л s ^2л “ е0^2л “ (£ “ 1) e0^2n e-1 q o г-l ql
=-------- -- - 5 cos 6 --—  -x
£+l2лг	e+12nr
£ —> 1	I	I	E — 1
(b)	Total bound charge is,------г q I --------й---йТТГ 2 л x dx - ------г q
47	e + 1 J 2ji(Z2 + x2)3	e + 1
0
3.90 The force on the point charge q is due to the bound charges. This can be calculated from the field at this charge after extracting out the self field. This image field is
E £-1 Я
image Ё+14ле0(2/)2
Thus,
F- e~1
£ + 1 16 Л Eo I2
303
3.91
Ер’
яК	,
-----з +--з—’ р m 1 4 л е0 Tj 4 л г2 е0
3.92
Ер-
Я ri
—- -3 , Р in 2
4 * Vi
where q" -	, q' - q" - q
In the limit I -* 0 k±jlE*_ я^ p 4 я e0 r3 2 л e0 (1 + e) r3’
Thus,
E.-^
in either part
p 2 л e0 (1 + e) r2
<p----------2________
2 л e0 (1 + e) r
________2________x f 1 in vacuum 2 л e0 (1 + e) r2 [ e in dielectric
j +---------т; P in 2
4 л e0 e r2 4 л e0 i\
->	4 r2
EB-----P m 1
p 4 я e0 r23
Using the boundary conditions,
Е1Я « e , Eu - E^
This implies
q - e « q" and q + e q' - e q"
So,
Then, as earlier,
, qt /e-l\ 1 2л/ \e + 1/ e
3.93 To calculate the electric field, first we note that an image charge will be needed to ensure that the electric field on the metal boundary is normal to the surface.
304
The image charge must have magnitude -
so that the tangential component of the electric field may vanish. Now, E - —-— " 4ле0
2 cos 0 = ----—т
2 л e0 e r
Then Pn - D - e0E » f - o' 2 ЛЕГ
This is the density of bound charge on surface.
the
3.94
Since the condenser plates are connected,
E1h^E2(d-h)^ 0
3.95
3.96
Given P » a r? where distance from the axis. The space density of charges is given by, p' = - div P = - 2a
On using.	div 7-	2
In a uniformly charged sphere,
E « -— or, 3 Eq
z* Po -* — r 3e0
•The total electric field is
£ = зГ	Po
Jt()	JtQ
1 Я-	P
= зГ P°Sr= "зГ ^Eo	JEq
—>
where p 6 r « - P (dipole moment is defined with its direction being from the -ve charge to +ve charge.)
The potential outside is	ж
1 (Q. Q
4 Л Eq r I r - d П j 4яе0г1 * 3 4
__Дд a ___________
where p0 » - — R p0 dr is the total dipole moment.
305
3.97	The electric field EQ in a spherical cavity m a uniform dielectric of permittivity e is related to the far away field E, in the following mannenjmagine the cavity to be filled др with the dielectric. Then there will be a uniform field E everywhere and a polarization P, given by-
P- <«-l)e0£
Now take out the sphere making the cavity, the_£lectric field inside the sphere will be P 3e0 p By superposition. EQ - -— « E 3e0
ог.Ёо» £ + |(e-l)£ = |(e + 2)£
3.98	By superposition the field E inside the bal^is given by Г Г P £'£»’3^
On the other hand, if the sphere is not too small, the macroscopic equation P « (e - 1) e0E must hold. Thus,
£ l+j(e-l) -£0 or,
Also	P- 3e0|^£0
3.99	This is to be handled by the same trick as in 3.96. We have effectively a two dimensional situation. For a uniform cylinder full of charge with chaige density p0 (charge per unit volume), the electric field E at an inside point is along the (cylindrical) radius vector r and equal to,
div £ * - -7- (rEJ « -2-, hence, Er« 7^“ й r dr r e0	r 2e0 I
Therefore the polarized cylinder can be thought of as two equal and opposite charge distributions displaced with respect to each other
E = 77— p rt- ~ p (ft-	p 6 r* -
2e0	2e0	2e0	2e0
—>
Since P « - p 6 r. (direction of electric dipole moment vector being from the negative charge to positive charge .)
p*
3.100 As in 3.98, we write E = Eo - -—
2s0
using here the result of the foregoing problem.
Also	(e - 1) e0E
——>
—*/ ₽ + 1 \	2 Ед	—*	f ««1 —*
So, £|-~4»£0, or, £“ -------------г and P-2ел^-у£о
121 u	e+1	ue+l
306
3.3	ELECTRIC CAPACITANCE ENERGY OF AN ELECTRIC FIELD
3.101	Let us mentally impart a charge q on the conductor, then
3.102	From the symmetry of the problem, the voltage across each capacitor, Aqp « §/2 and charge on each capacitor q « C § / 2 in the absence of dielectric.
Now when the dielectric is filled up in one of the capacitors, the equivalent capacitance of the system,
and the potential difference across the capacitor, which is filled with dielectric,
A /	< c* %	%
ф = eC ~ (1 + e) Ce “ (l-i- e)
But	qp a E
So, as ф decreases (1 + e) times, the field strength also decreases by the same factor
and flow of charge,At? « q' -q
s (1 + e) * 2*’ 2 *(e + l)
3.103 (a) As it is series combination of two capacitors,
1 dj + d}	~ ______Eq*______
C e0 ei 5 e0 e2	(d^/+(
(b) Let, о be the initial surface charge density, then density of bound charge on the boundary plane.
307
But,
S S e2 + eA J2 5
So,
Е0У(Е1-С2) e2 dr + ег
3.104	(a) We point the x-axis lowards right and place the origin on the left hand side plate. The left plate is assumed to be positively charged.
Since 8 varies linearly, we can write, e(x) = a + bx
where a and b can be determined from the boundary condition. We have e = ег at x = 0 and 8 = e2 at x = d,
/Sn 8-« \
Thus,	e(x) - e. + —-— x
I a I
Now potential difference between the plates
(b) D = I and P =	.
v ' S	S Se (x)
and the space density of bound charges is
p' - - divP - -
? (e2 - ei) S<fe2(x)
3.105	Let, us mentally impart a charge q to the conductor. Now potential difference between the plates,
ф+-ф-
J E dr
Ri

J 4 л e0 a/r Ri
Hence, the sought capacitance,
c=—. ф+-ф-
^dr= — lnP,/P r2 . 4ле0а z
q 4 л е0 а 4 л eq a q\nR2/RY ~ 1пЯ2/Я3
308
3.106	Let X be the linear chaige density then, £ - -----------------------------------------------------
(1)
and,
£ « 2o0/?2e2
The breakdown in either case will occur at the smaller value of r for a simultaneous breakdown of both dielectrics.
From (1) and (2)
ElmR1 ci w ^2ж^2е2» which is the sought relationship.
(2)
3.107
Let, X be the linear chaige density then, the sought potential difference,
Ф+-Ф-
X 2 Я Eq
I X . I	,
I "X"------«г+ I z-----------dr
J 2яе0е1г J 2яe0e2r \	*г
 ~ In R2/Rx + — in R^R2
I £1	e2
Now, as, E1R1e< E2 R2 e2, so
- £1Л1е1
2 Л Eq
is the maximum acceptable value, and for values greater than Е1 ep dielectric breakdown will take place,
Henc< Ae maximum potential difference between the plates,
— lnA2//?j+ —1пА3/Я2
£j	82
4
Er Rr In R2/Ri + — In R3/R>
Ф+ - <p_ = Er R} ex
2
e2
34QB Let us suppose that linear chaige density of the wires be X then, the potential difference, Ф+-Ф_ = ф-(-ф)ж 2 ф. The intensity of the electric field created by one of the wires at a distance x from its axis can be easily found with the help of the Gauss’s theorem,
E- —
2 я E0X b-e
,	Г г- » X , b — a
Then, ф « I E ax « ------In-------
J 2яе0 a a
Hence, capacitance, per unit length, 1 e 271E0
Ф+ w ф_ In b/a
309
3.109
3.110
3.111
The field in the region between the conducting plane and the wire can be obtained by using an oppositely charged wire as an image on the other side.
Then the potential difference between the wire and the plane,
2 я е0 г 2it£Q(2b-r)
, b
In —------
2b - a
Aqp « I E • dr
X 2 я e0
X
2 Я 8q
X 2 Я Eq
In — - -----
a 2 я Eq , 2b-a In ------
a
i 2b In —, as a
Hence, the sought mutual capacitance of the system per unit length of the wire = 2jte°
Aqp In 2b/a
When b » a, the chaige distribution on each spherical conductor is practically unaffected by the presence of the other conductor. Then, the potential qp^ (qp_) on the positive (respectively negative) charged conductor +^_ (__________M
4яе0еп I 4яЕ0еп1
Thus a; - qp =	—
2яееоя
and C « —— * 2яепея.
<P+ ~ Ф- °
Note : if we require terms which depend on p we have to take account of distribution
of charge on the conductors.
As in 3.109 we apply the method of image. Then the potentical difference between the +vely charged sphere and the conducting plane is one half the nominal potential difference between the sphere and its image and is
4 »-5<
Thus
—= 4яепд. for /» a. A qp u
310
3.112
(a) Since фх = qpB and qp2 = фл
The arrangement of capacitors shown in the problem is equivalent to the arrangement shown in the Fig.
and hence the capacitance between A and В is, C —	4- C2 +
(B)	From the symmetry of the problem, there is no P.d. between D and E.. So, the combination reduces to a simple arrangement shown in the Fig and hence the net capacitance,
3.113
c -£+£-c c° 2 + 2
(a)	In the given arrangement, we have three e05 capacitors of equal capacitance C = -j- and the first and third plates are at the same potential.
Hence, we can resolve the network into a simple form using series and parallel grouping of capacitors, as shown in the figure.
Thus the equivalent capacitance
_ (C + C)C	2
0 (C + C) + C 3
311
(b)	Let us mentally impart the charges +q and -q to the plates 1 and 2 and then distribute them to other plates using charge conservation and electric induction. (Fig.).
As the potential difference between lhe plates 1 and 2 is zero,
4i 42 4i n (	e0S)
С С C	d
or,
42" ^ 41>
The potential difference between A and B9
<P = Фл " <Рв = 42/c
Hence the sought capacitane, £ 41 + 42	3 4i 3 3jeqS
0 “ Ф “ q2/C ~ 2^/C *2 2d
3.114	Amount of charge, that the capacitor of capacitance Cr can withstand, qr = Cx Vx and similarly the charge, that the capacitor of capacitance C2 can withstand, q2 » C2 V2. But in series combination, charge on both the capacitors will be same, so, gmax, that the combination can withstand « Cx Vx, as Cx V1 < C2 V2, from the numerical data, given.
Now, net capacitance of the system,
Ci C2
°" c1 + c2
fl,	G	/ Ci )
and hence,	^^7^° У41 + 4 = 9kV
3.115	Let us distribute the charges, as shown in the figure.
Now, we know that in a closed circuit, - Дф = 0
-So, in the loop, DCFED, 4i 42	42
n Ft M 1 1 ?
Again in the loop DGHED,
91	91 + 92 fc
c~+~c—
c-1 C2
D
C
E
Ci
(?2 -|-
-(Wz)
Ci
cz^
---
F
(1)
(2)
H
312
Using Eqs. (1) and (2), we get
«2
Now,
“92	£
Фл-Фв- ~- ТГ7"
C2 V2/Cl
1	3 Ci
Ci4 + c22
or,
______§_______
T)2 + 3 T) + 1
10 V
± 3 El
^2	^2

<Pa-<Pj-
3.116	The infinite circuit, may be reduced to the circuit, shown in the Fig. where, Co is the net capacitance of the combination.
o 1	1 1	C
C + Co C Cq		| p-‘ —-,
Solving the quadratic,	~ J. г
CC0 + C02-C2« 0,	*
we get,
('M
Co------z----C, taking only +ve value as Co can not be negative.
3.117	Let, us make the charge distribution, as shown in the figure.
Now, <РЛ-ФЯ-^--1 + ^-
12	Ct	C2
ci + c2
Непсе, voltage across the capacitor C\
- -3-. Cr
(Фл-Фв)+
c^c2
10 V
and voltage across the capacitor, C2
я (Фл-.Фв).^.-с Ш 5V С2 с1 + с2 C1
А
3.118	Let > then using - Дф « 0 in the closed
circuit, (Fig.)
or,
(Ci -i- C2)
D
Cz
c
313
Непсе the P.D. accross the left and right plates of capacitors, q _	“ ?i) ^2
,c1 + c2
and similarly
-q
ф2 c2 c1 + c2
3.119	Taking benefit of tBe foregoing problem, the amount of charge on each capacitor
lgl~ - C.iC,
3.120	Make the charge distribution, as shown in the figure. In the circuit, 12561.
- Aqp = 0 yields
NT	%2
Now <PA - <₽b -
9i	9i	^C3C4
C4 C3	C3 + C4
and in the circuit 13461,
#2	#2	? Ci C2
— -i- — - t = 0 or, q~ = —----------—
C2 сг	Cr -i- c2
41 C3
It becomes zero, when
С. C3 (C^-C^CJ- 0. or -1--2
c2 C4
3.121 Let, the charge q flows through the connecting wires, then at the state of equilibrium, charge distribution will be as shown in the Fig. In the closed circuit 12341, using -Aqp= 0
_<£^> » » „ C1	°2 q	-HCV-«)		
		C2	
		
nr Z1 - 	—	 - П.ЛА тГ					
(1/C1 + 1/C2 + 1/C3) 3.122 Initially, charge on the capacitor or C2,		I к 	c3
		-г
as they are in series combination (Fig.-a)
|£iq
Ci + С?2
314
when the switch is closed, in the circuit CDEFC from - Дф « 0, /(Fig. b )
0 or q2~ C2%	(1)
C2
And in the closed loop BCFAB from - Дф = 0
И
From (1) and (2) qY = 0
Now, charge flown through section 1 =	+ q2) - 0 - C2 §
and charge flown through section 2 = - qY- q = -
C y + C2
3.123 When the switch is open, (Fig-a) 2?CxC2 q°~ c, + c,
1
+Яо -r
-rC2
2
and when the switch is closed, ?i“ Kci and q2 = %C2
Hence, the flow of charge, due to the shortening of switch,
Гс.-с2
through section 1 = qx - qQ = C
through the section 2 = - q2 - (qQ) = % C
= -24цС
Cj - C2
2 Cj + C2
= - 36 цС
3
2
and through the section 3 = q2 - (q2 -	- 0 = % (C2 - Cx) = - 60 цС
315
3.124
First of all, make the charge distribution, as shown in the figure.
In the loop 12341, using - Аф « 0
Similarly, in the loop 61456, using - Аф * 0 4г 4г “ 4i F+~c~"^= 0 C2 c3
From Eqs. (1) and (2) we have
^2 C2 - £1 Cj
c, c,
(1)
(2)
3
Hence,
4г " 4i
C3
?2 C2 - 11 Cl ci + c2 + c3
3.125
In the loop ABDEA, using - Лф = 0 fc 4i 4i + 42 fc n
-S'c/V*’’
Similarly in the loop ODEF, О 4i + 4г	4г n
“7— + ?i“S2 + ^~= 0
C1	U2
Solving Eqs. (1) and (2), we get,
§2 ^2 ~ §1 c2 - C3 +§3 c3
4i + 42=	-----------
Now, (Pi - cp0 =
C? c2 c/c/
<Pi = -	, as (<p0 = 0)
(1)
(2) p
1______I iMl... D
Cl
^(C2 + C3)- ^C2-l3C3
Cl + C2 + C$
A . . -	I2 + c3) - li Ci -|3 c3
And usmg the symmetry, ф2 =
Ci + C2 + C3
and
?з (Ci + C2) - Ci - c^
<Рз ~
Cl + C2 + C3
The answers have wrong sign in the book.
2
3
c5
Б
3.126 Taking the advantage of symmetry of the problem charge distribution may be made, as shown in the figure.
In the loop, 12561, - Аф « 0
316
or
or
92	92 ~ 91 _ 91 _
С2 + С3 Cj
9i _	1 (^з + ^2) z. .
д2 С2 (Cj + С3)
Now, capacitance of the network,
6
4г л
3.127
c =
° Фа - <PB <h/C2 + 91/C1
(1 + q/qj ' 1 ?i .^2	^2^1
(2)
From Eqs. (1) and (2)
Ст
A
7

2CrC2 + C3 (Cj + C2) Co = r
з
C2
Ml
T Сз
Mr
2 fcJJVs
в
.	.	.	91 92	,
(a) Interaction energy of any two point charges qr and q2 is given by 4я where r
is the separation between the charges.

г
+7
n
a.
-4

Hence, interaction energy of the system, 2
17 = 4—2----+ 2-------
4 л e0 a 4 л e0 (V2 a)
C7b= 4 ~	+2-----^—7=—
4 л e0 a 4 л £0 (V 2 «)
Ч2
and
VI g2
U = 2	____2?2	_ _
c 4яе0а 4яе0« 4ле0(Т2а) 4ле0а
317
3.128 As the chain is of infinite length any two chaige of same sign will occur symmetrically to any other charge of opposite sign.
So, interaction energy of each charge with all the others,
4	1	1	1
1 —	~	~	~	T
2	3	4
U = -2 —*--
4 Л80Я
up to 00
1 2	1 3
But	ln(l+x)=x- — X + jX ....UP to 00
and putting x « 1 we get In 2 - 1 - — + — +.up too©
From Eqs. (1) and (2),
u. -2^‘"2.
4яе0д
(1)
(2)
3.129	Using electrical image method, interaction energy of the charge q with those induced on the plane.
cz=.....-i-_-0—
4 я e0 (21)	8 я e01
3.130	Consider the interaction energy of one of the balls (say 1) and thin spherical shell of the other. This interaction energy can be written as J*dyq
4i
4 ЛЕ0Я
p2 (r) 2 я r2 sin 0 d 0 dr = j
о
p2 (r) qr r2 sin 0 d 0 dr
2 e0 (Z2 + r2 + 2Zr cos 0)1/2
Z + r
q, r
- ^—dr-2rf>2(r) -2 Z Eq I
“ 7777431
*4 JL Cq I
Hence finally integrating
°int"	where> f 4nr2p2(r)dr
H Я Ел * 0
3.131 Chaige contained in the capacitor of capacitance is q = cp and the energy, stored in it :
Ui~ ктг* 4С1Ф2
‘ 2Ct 2 1Y
Now, when the capacitors are connected in parallel, equivalent capacitance of the system, C =	+ C2 and hence, energy stored in the system :
318
С/ ф2
£Л= ---—----
f 2(С1 + С2)
as charge remains conserved during the process.
So, increment in the energy,
CiVr i j_\ 2 I Cx + C2 ~ Cj I
- C2 Cx ф2 2(C1 + C2)
- 0-03 mJ
3.132 The charge on the condensers in position 1 are as shown. Here q (7 + #o C	Co C + Cq
/ 1	1\	C(C + C0)
a"d	»•	C0 + 2C
c4	cco?
Hence’	f/=c^Tc and

After the switch is thrown to position 2, the charges change as shown in (Fig-b).
A charge q0 has flown in the right loop through the two condensers and a charge qQ through the cell, Because of the symmetry of the problem there is no change in the energy stored in the condensers. Thus
H (Heat produced) = Energy delivered by the cell CC0£2 =	= c0 + 2C
3.133 Initially, the charge on the right plate of*the capacitor, q = С -%2) ап^ finally, when switched to the position, 2. charge on the same plate of capacitor ;
q'-C^
So,	Aq ** C ^2
Now, from eneigy conservation,
Д£7 + Heat liberated « A where AU is the electrical energy.
319
| С	1С	- ^)2 + Heat liberated = A q
as only the cell with e.m.f.	is responsible for redistribution of the charge. So,
1	0
C ^2 — — C §2 + Heat liberated « C Ц2
t 2
Hence heat liberated - j C §2
3.134
Self energy of each shell is given by ^5 where qp is the potential of the shell, created
only by the charge q, on it.
Hence, self eneigy of the shells 1 and 2 are :
2	„2
q?
Ж - о  n and W2 - Q 2 n 1 8яе0£1	2 8ле07?2
The interaction energy between the charged shells equals charge q of one shell, multiplied by the potential qp, created by other shell, at the point of location of charge q.
So,	= q. -------— = --------—
12	14ле07?2 4ле0^2
Hence, total enegy of the system, U = Wr + W2 + W12
1
4 я e0
$2	#2
27?1 + 2jR2+ R2
3.135
Electric fields inside and outside the sphere with the help of Gauss theorem :
£1- И УП2<Г^ £)>£2- T?r4(r>£) 4ле0л	4яе0/
Sought self energy of the ball
Wq + W2
R
Hence,
f ₽ £2
I 4)^1
J 2
0
/£ F 2
4)^2
——
R
u- —— 4 я e0 5 R
4 nr2dr-	0(7 + 1
8яе0£ 15

1
5
3.136
2	2
t-£E0£ 4 л r dr, for a spherical layer.
j 1	2	11
(a)	By the expression I — totE dV = I —
To find the electrostatic eneigy inside the dielectric layer, we have to integrate the upper expression in the limit [a, b] b
U- |e0£
2
—9 | 4 я r2 dr oer J
./[1,11
8 я e0 e a
27 mJ
b
320
3.137
As the field is conservative total work done by the field force, Afd- ut~uf= ^g(<pl-«p2)
1 <72 Г 1 _ 1 I q2 Г_11_ 1
2 4ле0 2?x R2 8ne0 Rr R2
3.138
Initially, energy of the system, Ц. = Wr + Ж12 where, is the self energy and W12 is the mutual energy.
So, U- L S + ™
1	2 4 я£0А1 4 я£0А1
and on expansion, energy of the system, uf- wl + wu
1 q2 ggo -------2---+-----------
2 4 л e0 R2 4 л e0 R2
Now, work done by the field force, A equals the decrement in the electrical energy,
i.e.
A= (Ц-^) =
4 (gp + g/2) /1
4лЕ0 11?!
A2j
Alternate : The work of electric forces is equal to the decrease in electric energy of the system,
A - Ut-Uf
In order to find the difference (/. - Up we note that upon expansion of the shell, the electric field and hence the energy localized in it, changed only in the hatched spherical layer consequently (Fig.).
*2
ui- Ur ^^(E2-E2)-4nr2dr
where Er and E2 are the field intensities (in the hatched region at a distance r from the centre of the system) before and after the expansion of the shell. By using Gauss’ theorem, we find
_ 1 + % _ 1 %
= ---------and E2 = ----------у
1	4 л e0 r2 2	4 л e0 r2
As a result of integration, we obtain
. q (g0 + g/2) /1 i \
A = ----------- I ______ I
4ле0 11?! 1?2I
321
3.139
3.140
Energy of the charged sphere of radius r, from the equation
1	1 q q2
2	2 4 л e0 r 8 л £0 r
If the radius of the shell changes by dr then work done is
4x,r2Fudr = -dU = ^2/8ле0г2
Thus sought force per unit area,
F -	,	<4 я f2a^2	—
u 4 л (8 л e0 г2) 4лг2х8ле0г2 2e0
Initially, there will be induced charges of magnitude -q and +q on the inner and outer surface of the spherical layer respectively. Hence, the total electrical energy of the system is the sum of self energies of spherical shells, having radii a and b, and their mutual energies including the point charge q,
1	2 4л е0Ь 24л e0«
„ q2 f1 C7 • = —4  — -1 8 л e0 о
Finally, charge q is at infinity hence, Uf = 0
Now, work done by the agent = increment in the energy
3.141
~gg + gg.....+ Z-gg „
4яе0а 4ле0Ь 4ле0Ь
1' a
or
= Uf- U =	—
f 1 8 л e0
a b
(a) Sought work is equivalent to the work performed against the electric field created by one plate, holding at rest and to bring the other plate away. Therefore the required work, A agent = qE {X2-Xl),
where E « -— is the intensity of the field created by one plate at the location of other. 2 e0
2
Alternate : A ext = AU (as field is potential) 2	2
____g_ у _ g * = .g— (x _ у } ~ 2e0S 2 2s05	2£oS( 2 J
(b) When voltage is kept const., the force acing on each plate of capacitor will depend on the distance between the plates.
So, elementary work done by agent, in its displacement over a distance dx, relative to the other,
So,
dA = -Fdx
But,

S a(x) and
e0V oW = —
l2
Hence,
. f,. fl SV2.
A = J dA = J -e0—j-dx =
2
*1 X2
322
Alternate : From eneigy Conservation,
cell + agent
or
( aS ^cdl
So
1 £0 у2	1 £0 $ у2 Д fo£ £0*
2 Xj 2 х^	X2 x^
(4f-q)v - (crc^v2)
^v2ji _ t a«en‘“ 2 X1 x2
V2 +A v + л agent
3.142
(a) When metal plate of thickness is inserted inside the capacitor, capacitance of the Eq ‘S system becomes Cn «	------
Now, initially, chaige on the capacitor, qQ - CQ V
Eq S Finally, capacitance of the capacitor, C - —7-a
As the source is disconnected, charge on the plates will remain same during the process. Now, from energy conservation, иГи^АчаЛ 1 ?o2 2 c :
2
e0SV d (1 - П)
or,
t (as cell does no work) 1 ?o2 2
Co “ Aa«ent
Hence A
igBOt
1Г eosv 2 d(l-n)
1(1 - n)'
c c
1 CV2n . _ , —-----!r« 1.5 mj
2(1-Л)2
(b)	Initially, capacitance of the system is given by,
C e
C° -	—~j~’g	*s caPac^tance °f capacitors in series)
So, charge on the plate, q0 » Co V
Capacitance of the capacitor, after the glass plate has been removed equals C
From energy conservation,
A aoent = Uf-U. agent j 1
" 2*0
0.8mJ
2 [e-n(e-l)]2
’ C Co
3.143 When the capactior which is immersed in water is connected to a constant voltage source, it gets charged. Suppose o0 is the free charge density on the condenser plates. Because water is a dielectric, bound charges also appear in it Let 0' be the surface density of bound charges. (Because of homogeneity of the medium and uniformity of the field when we ignore edge effects no volume density of bound charges exists.) The electric field due o0	0'
to free charges only —; that due to bound charges is — and the total electric field is £o	£o
°o
—. Recalling that the sign of bound charges is opposite of the free charges, we have EE0
323
ао ао а'	, (е -1 \
— =-----------or, а = I-------------- ап
ее0 е0 ео	к е /
Because of the field that exists due to the free charges (not the total field; the field due to the bound charges must be excluded for this purpose as they only give rise to self energy effects), there is a force attracting the bound charges to the near by plates. This force is
1 , (e - 1) o02
—(J --- = —----- U per umt area
2 e0 2ee0
The factor j needs an explanation. Normally the force on a test charge is qE in an electric field E. But if the chaige itself is produced by the electric filed then the force must be constructed bit by bit and is
F-J* q(E')dE'
0
if	q (E ') а E ' then we get
F-\q(E)E
This factor of ~ is well known. For example the energy of a dipole of moment p*in an
—*	—> —*
electric field Eo is -p • Eo while the energy per unit volume of a linear dielectric in an electric field is - i-P • Eo where P is the polarization vector (i.e. dipole moment per unit volume). Now the force per unit area manifests ifself as excess pressure of the liquid.
V ao
Noting that	-= = —
d ee0
We get
eoe(e - 1)V2
Ap =-------ж--
2?
Substitution, using e  81 for water, gives Ep - 7-17 к Pa = 0-07 atm.
3.144 One way of doing this problem will be exactly as in the previous case so let us try an alternative method based on energy. Suppose the liquid rises by a distance h. Then let us calculate the extra energy of the liquid as a sum of polarization energy and the ordinary gravitiational energy. The latter is
1	1	9
-h- pg • SA =-pgSA2
If a is the free charge surface density on the plate, the bound charge density is, from the previous problem,
, e - 1 а =-------а
e
This is also the volume density of induced dipole moment i.e. Polarization. Then the energy is, as before
1	, „	-1	, a - (e -1) a2
“ 2 ’ ° £° ° 2 ° e0 ~ 2e0e
324
and the total polarization eneigy is
с / ь \ (e - 1) cr2
- S (a + h)	.1—
2e0e
Then, total eneigy is
[Z(A) = -5(a+A)lL2_U2! + lpg5A2
This gives
The actual height to which the liquid rises is determined from the formula ^-CZ'(*)-0 an
/t„ (e-l)a2 2e0 e pg
2
We know that energy of a capacitor,?/ «	.
3.145
3.146
Hence, from F =	we have, F =	/ C2
d* «-Const.	2 dx /
d
Now, since d « R, the capacitance of the given capacitor can be calculated by the formula of a parallel plate capacitor. Therefore, if the dielectric is introduced upto a depth x and the length of the capacitor is /, we have,
2 о0еЯх 2nR e0(1-x) c = d
From (1) and (2), we get,
Fx= e0(e-l) —j—
When the capacitor is kept at a constant potential difference V, the work performed by the moment of electrostatic forces between the plates when the inner moveable plate is rotated by an angle dqp equals the increase in the potential energy of the system. This comes about because when charges are made, charges flow from the battery to keep the potential constant and the amount of the work done by these charges is twice in magnitude and opposite in sign to the change in the energy of the capacitor Thus
(2)
z dq> 2 dq)
Now the capacitor can be thought of as made up two parts (with and without the dielectric) in parallel.
‘  a;"-------------Й--------
1 2
as the area of a sector of angle ф is ф. Differentiation then gives

(e-l)£oR2V2 4d
The negative sign of Nz indicates that the moment of the force is acting clockwise (i.e. trying to suck in the* dielectric).
325
3.4 ELECTRIC CURRENT
3.147 The convection current is
T. dt
here, dq = к dr, where к is the linear charge density.
But, from the Gauss’ theorem, electric field at the surface of the cylinder,
E =	—
2 л e0 a
Hence, substituting the value of X and subsequently of dq in Eqs. (1), we get 2E я en adx — dt
~	„ dx
« 2heqE av, as -p- v
3.148 Since d « r, the capacitance of the given capacitor can be calculated using the formula for a parallel plate capacitor. Therefore if the water (permittivity e) is introduced up to a height x and the capacitor is of length Z, we have,
ee0-2 я rx e0 (I - x) 2 яг e0 2 яг
C-—T~ +----------d---------
Hence charge on the plate at that instant, q - CV
Again we know that the electric current intensity,
I.
dt
d(CV) dt
Vt02xr d(ex + l-x) V2xrt0 fa —----------Л----------
So,
2 я r e0 (e - 1) V ------------------v
041 ц A
3.149 We have, Rt = RQ (1 + ar),(l)
where Rt and RQ are resistances at f C and 0° C respectively and a is the mean temperature coefficient of resistance.
So,	Rr = Ro (1 + o^ t) and R2 = r] Ro (1 + a21)
(a)	In case of series combination, R = YRi
so	K= Ях+Я2= Яо [(1 + tq) + (<4 + т] a2) Г]	(1)
= /?o(l + n)
«1 + 1102
1 +T]
(2)
1 + 2
326
Comparing Eqs. (1) and (2), we conclude that temperature co-efficient of resistance of the circuit,	04 + yj <x2
cx = —--------
1 +n
(b)	In parallel combination
R =
Яо (1 + сц t) Яр n (1 + «20 Яр (1 +040 + 11^0 (! +a2*)
R' (1 + a' r), where R' «
ПЯр
1 + Y|
Now, neglecting the terms, proportional to the product of temperature coefficients, as being very small, we get,
J	° ’	r| 04 + a2
3.150 (a) The currents are as shown. From Ohm’s law applied between 1 and 7 via 1487 (say)
(b)	Between 1 and 2 from the loop 14321,
Zj R = 2/2 R +13 R or, Ij = Z3 + 2/2
From the loop 48734,
(/2 - /3) R + 2 (/2 - /3) R + (/2 - /3) R = /3 R.
4
or,	4 (/2 - /3) = /3 or Z3 » —12
1	14 т
so	A=yA
24	14
Then, (/1 + 2/2)Яеч =
327
7
ог	eq "" 12	1
(с)	Between 1 and 3
From the loop 15621
I2R= I.R+^R or, I2~ 3^-
Then, (Z1 + 2Z2)^- 4I,Req
- J2R+I2R- l^R 3
Hence, R.a = —R . «7	4
3.151 Total resistance of the circuit will be independent of the number of cells,
(ЛХ + 27?)Л = ---------
x RX + 2R+R
or,	R2 + 2RRX-2R2 = 0
On solving and rejecting the negative root of the quadratic equation, we have, Rx = R(V3 -1)
3.152	Let Ro be the resistance of the network,
Я0Л2
then, Ru=	ot RQ-R0R1-RiR2 = 0
Ao +K2
On solving we get,
R i f ч / Ry | Ao = V 1+v 1+4-2- - 6Q
1
328
3.153	Suppose that the voltage V is applied between the points A and В then У-Ж-Vo,
where R is resistance of whole the grid, Z, the current through the grid and Zo, the current through the segment AB, Now from symmetry, 1/4 is the part of the current, flowing through all the four wire segments, meeting at the point A and similarly the amount of current flowing through the wires, meeting at В is also 1/4, Thus a current 1/2 flows through the conductor AB, i.e.
Л)
z
2
Hence,
*0
2

3.154	Let us mentally isolate a thin cylindrical layer
of inner and outer radii r and r + dr
respectively. As lines of current at all the points of this layer are perpendicular to it, such a layer can be treated as a cylindrical conductor of thickness dr and cross-sectional area 2 л rl. So, we have,
,D dr dr
dR = p -  - = p -—;
H S (г) H 2л rl
and integrating between the limits, we get,

X-z In —
2л/ a
3.155	Let us mentally isolate a thin spherical layer of inner and outer radii r and r + dr. Lines of current at all the points of the this layer are perpendicular to it and therefore such a layer can be treated as a spherical conductor of thickness dr and cross sectional area 4 л r2. So
dR = p
dr
4 л r2
(1)
And integrating (1) between the limits [a, ft], we get,
Г b
Now, for Ь oo, we have
R =
_JL_ 4 л a
3.156
In our system, resistance of the medium R =
p Г1 1
4 л a b
where p is the resistivity of the medium

The current
<p_____
_g_[l _1 4 n a b
329
ж,	-dq d(Cw) dq>
Also, i«	= - C , as capacitance is constant.
So, equating (1) and (2) we get, ______________________________T_____ p Г1 1' 4 я a b
(2)
dt
or,
a b
Ср[1Г 4 я
or,
At 4 nab in n ж --------
1 Cp(b-a)
Hence, resistivity of the medium, _	4 я Ar ab
P C (b - a) In T)
3.157 Let us mentally impart the charge +q and -q to the balls respectively. The electric field strength at the surface of a ball will be determined only by its own charge and the charge can be considered to be uniformly distributed over the surface, because the other ball is at infinite distance. Magnitude of the field strength is given by,
<1
4 я e0
£-
1 Q
So,	current density j «---------—«
P 4 я e0 a2
and electric current
I~ f jS = -------2—г4лЛ -3-
J	p 4 я e0 a	P eo
But, potential difference between the balls, Ф+-Ф_« 2 —— + 4леоа
Hence, the sought resistance,
<p+ - cp 2g/4 я e0 a p
Z q/p e0 2 na
3.158 (a) The potential in the unshaded region beyond the conductor as the potential of the given charge and its image and has the form 7 1 1 \ qp = Al — - — f2 )
where rp r2 are \he distances of the point from the charge and its image. The potential has been taken to be zero on the conducting plane and on the ball
cp « A
£

330
So A « Va. In this calculation the conditions a « I is used to ignore the variation of cp over the ball.
The electric field at P can be calculated similarly. The charge on the ball is
Q - 4ле0Уд
л	г Va - A 2alV
and	Ер « —у 2 cos0 « —у—
г	г
1	2alV
Then j = —E ® —T- normal to the plane.
P	pr3
(b) The total current flowing into the conducting plane is
о
2 nxdxj
(On putting у = x2 + I2 )
2 я alV Г dy _ 4naV p J y3/2~ p t
Hence
3.159 (a) The wires themselves will be assumed to be perfect conductors so the resistance is entirely due to the medium. If the wire is of length L, the resistance R of the medium is a j- because different sections of the wire are connected in parallel (by the medium) rather than in series. Thus if Rr is the resistance per unit length of the wire then R « Rr/L. Unit of R t is ohm-meter.
The potential at a point P is by symmetry and superposition
(for I » a)
V A i a
Then <px = — = — In -
(for the potential of 1)
331
or,	A - - V/ln -a
and	’ - - 2 In l/a ln
We then calculate the field at a point P which is equidistant from 1 & 2 and at a distance r from both :
Then	V / 1 \ £ « _ , , z 1 x 2 sinG 21n//a r 1 . W 1 2 In l/a
and	T	F 1 V 1 °	p 21nl/a r2
(b) Near either wire	Е_-У—к llnl/a a
and	T	r 1	V J = OE « — -	; p 21п//д
Then	v	у I = i = L±- - J 2naL R
Which gives	R< - £ In l/a 1	Л
3.160	Let us mentally impart the charges +# and -q to the plates of the capacitor. Then capacitance of the network,
n q eeo
<P Ф
Now, electric current,
i=	as ft	(2)
Hence, using (1) in (2), we get,
3.161	Let us mentally impart charges +q and -q to the conductors. As the medium is poorly conducting, the surfaces of the conductors are equipotential and the field configuration is same as in the absence of the medium.
Let us surround, for example, the positively charged conductor, by a closed surface 5, just containing the conductor,
then,	R =	= — -----= -—; as j f f E
‘ finds’
a.	r £
and	C =	= ---------
Ф Ф
ee0
So,	RC = — = p ee0
a °
332
3.162	The dielectric ends in a conductor. It is given that on one side (the dielectric side) the electric displacement D is as shown. Within the conductor, at any point A, there can be no normal component of electric field. For if there were such a field, a current will flow towards depositing charge there which in turn will set up countering electric field causing the normal component to vanish. Then by Gauss theorem, we easily derive a « Dn « D cos a where a is the surface charge density at A.
The tangential component is determined from the circulation theorem
j) E-dr*= 0
It must be continuous across the surface of the conductor. Thus, inside the conductor there is a tangential electric field of magnitude, Dsina ж .
-------at A .
eoe
This implies a current, by Ohm’s law, of D sin a
J = -------
eoep
3.163 The resistance of a layer of the medium, of thickness dx and at a distance x from the first plate of the capacitor is given by,
dR =
1 dx a (x) 5
(1)
Now, since a varies linearly with the distance from the plate. It may be represented as, ° « <?! + —-— x , at a distance x from any one of the plate.
From Eq. (1)
or,
1 dx	d , cf2
5 J	(a2 - °i\	(°2 ” ai) ai
о ai+ —1— *
x I /7 I
Hence,
. V l~ R
SV(o2-O1)
---------= 5 nA °2 din — ai
3.164 By charge conservation, current j, leaving the medium (1) must enter the medium (2). Thus
Д cos 04= J2 cos «2
Another relation follows from
&lt ж >
333
nR2
p2/
and that in 2 is E? « —т
xR2
Applying Gauss’ theorem to a small cylindrical pill-box at the boundary.
Pi1 P21 odS
------T aS +---x- aS * ---- itR itR eo
a “ e0 (P2 ” Pl' „ It К
and charge at the boundary* e0 (p2 - рг)/
Thus,
3.166
We haverE1 dx + E2 d2 « V and by current conservation
— Ej- —E2
Pi P2
Pi
Thus, Ex =	-	, ,
Pi “1 + P2 &2
c P2V
Е2ж —	~
Pl "1 + P2 “2
di dz

At the boundary between the two dielectrics, 0 « D2 “	“ eo e2 ^2 ~ eo ei ^1
e0V
o Л + o Я (C2 P2 " 61 Pl)
Pl «1 + P2 a2
3.167	By current conservation
E(x) ж Е(х)-ь JE(x) m dE(x) p (x) “ p (x) + d p (x) “ d p (x)
This has the solution,
E(x)- Cp(x)-
334
Непсе charge induced in the slice per unit area
do = e°£ [ {e (x) + de (x) } {p (x) + d p (x) } - e (x) p (x) ] = e0 jd [e (x) p (x) ]
Thus,	d Q = e0 Id [ e (x) p (x) ]
Hence total charge induced, is by integration, 2 = e0Z(e2p2-e1p1)
3.168	As in the previous problem
£ (x) = С p (x) - C (p0 + px x)
.	,	(П -1) Po
where	Po + Pi = П Po or, px - -----
d /I Л — 1\	1
C p (x) dx = C p0 d 1 +	— = — C p0 d (r| + 1)
о	V '
Thus volume density of charge present in the medium = s£ = ^dE{x)/dx
2e0V (n-l)p0 _ 2e0V(n-l)
Po d (n + 1) X d (n + 1) d2
3.169	(a) Consider a cylinder of unit length and divide it into shells of radius r and thickness dr Different sections are in parallel. For a typical section,
, ( 1 \ 2л r dr 2 л r3 dr
(a/r2) a
Integrating,	1	лЯ4	s2
		2 a	2л a
or,	_ 2ла 1 s2	, where	5= лЯ2
(b) Suppose the electric filed inside is Ez = Eo(Z axis is along the axiz of the conductor). This electric field cannot depend on r in steady conditions when other components of E are absent, otherwise one violates the circulation theorem
The current through	J> E • dr = 0 a section between radii ( r + dr, r) is 1	a E 2л^г п E - 2лг dr ac/r2	ж
Thus	. t » 3. £	xR'E I - 1 2лг dr -J*	a	2« 0
Hence	2<Х‘Л1	? E = —y— when S = лк s2
335
3.170	The formula is,
9= CV0(l-e-^c)
or,	уо(1 _е"'/дс) or, ~-l-e~,/KC
C	vn
Vo
Hence, t = RC In —= R C In 10, if V = 0-9 Vo. Vo ” у
Thus t = 0.6 p,S.
3.171	The charge decays according to the foumula л	X. z» -t/R c
Q ~ Qq e
Here,	RC - mean life = Half-life/ln 2
So,	half life= T= RC In2
ee0 A	od
But,	C= —3—,^=Er
a	A
Недсе,	p = ——— = 1.4 x 1013 Q • m
ee0ln2
3.172	Suppose q is the charge at time t. Initially q = C at t = 0.
Then at time r,

But,i =
sign because charge decreases)
So C dt
or, dt	R
or,	q=91+Ae-^RC
n
A = C £ 11 - -|, from q = C % at t = 0
к W
Hence,	сИ-+(1-
M ’V /
Finally,	i-
л’	dt R
3.173	Let r « internal resistance of the battery. We shall take the resistance of the ammeter to be = 0 and that of voltmeter to be G.
Initially,V- l-Ir, 1=
336
So,

(1)
3.174
3.175
After the voltmeter is shunted
(Voltmeter)
R + G
and -» T| —(Ammeter)
R G r + G
From (2) and (3) we have
л
r + G
From (1) and (4)
(2)
(3)
R
£
— = r + G - T]r or G = T|r
Then (1) gives the required reading
T| T| + 1
Assume the cunent flow, as shown. Then potentials are as shown. Thus, Ф1 = фх - IRy + 5i - IR2 -r ?1~?2 “ Ry+R2 ф2 = (Pi - IRy + 51
or,
And

Rr
(4)

2
£
-^RP^R^
= -(M2 +	)/(/?! + R2) =
Let, us consider the current i, flowing through the circuit, as shown in the figure.
Applying loop rule for the circuit, - А ф = 0
— 25 +	+ iR2 + iR e 0
HR^R^+R)- 25
i. .	..
R + Ry + R2
So,
X
-4 V
f-IRi+b-IRz
or,
or,
Now, if
Ф1 - <p2 = °
- § + iR{ - 0 2^
0Г’ R +RX +R2 " and 2/?1’‘ Я2+Л+Л1
or, R* 2?! - R2> which is not possible as R2 > Ry
Thus,	Ф2 ~ Фз ж - § +1K2 - 0
337
or,
So,
2lR2
R + Rl + R2m 5
R - R2 - Rp which is the required resistance.
3.176
(a)
Cunent, i- — - a> as aR (g*ven)
3.177
3.178
3.179
(b)
As
Ч>А-фв = n^-niR= naR - naR = 0
the capacitor is fully charged, no current flows through it So, cunent
^2 ~	Z C. C. V
sj«;("s ^>6,)
And hence, фл - <рв я - ?2 + 1 ^2
.fe-W.-o-sv я1+я2
Let us make the current distribution, as shown in the figure.
Current i - --— (using loop rule)
IL Ry
So, current through the resistor R
Л__________R2
Rx R2 R^ + R2
Л, + Я2 |/?2

м. — —	~~ —~	1 2
R Rr + R R2 + Rr R2
and similary, current through the resistor R2,
4Ri
________
Ry Ry Ry + Ry R +	.£ - 1	2
+ ^2
/ — X
Total resistance- ——

-я0Г/'х
i
Ъ
Ri
rr1+r1r2+rr2 °‘8a
R.^- +___I-
0 + Y Я xRRq IR+xRq
° Vo
Ro
V
&

xR
I "lR+xR0
R
338
Т7 Т7
Then V « Vn —----—-
0 IR+xRq
i _£
~ l+ xR0 + lR
For
Voy
3.180 Let us connect a load of resistance К between the points A and В (Fig.)
From the loop rule, Д cp » 0, we obtain iR‘^~ i.R, (1)
and i R *	- (i-ii)R2
or i(R+R2} - S + hR2 (2)
Solving Eqs. (1) and (2), we get
11^1 + §2^2 I r ^1^2
1 ~ rx+r2 / ry + r2 ж r + rq
^]Rl + ?2^2	^1^2
where -	..	... and Ro - _ - -
+ /<2	/vj » /<2
Thus one can replace the given arrangement of the cells by a single cell having the emf and internal resistance Ro.
3.181
Make the current distribution, as shown in the diagram.
Now, in the loop 12341, applying - Д qp = 0
iK + i^ + ^-O	(1)
and in the loop 23562,
1Я-^ + (*-ч)Я2в 0	(2)
Solving (1) and (2), we obtain current through the resistance Я,
R Я j + R R2 + Я । R2
and it is directed from left to the right
3.182 At first indicate the currents in the branches using charge conservation (which also includes the point rule).
In the loops 1 BA 61 and B34AB from loop rule, - Д ф » 0, we get, respectively ”	+	” £\)^2 +	“ h = 0 iR3 + 11 - ('-‘1) ^2 +	~ 0 On solving Eqs (1) and (2), we obtain Ch - &R3 + R2 + У nnz	the (1) (2)	6	A			i 4		
			\R1	к				--6 \Ri
								
Я^Я2 + R2R. + R^ R^i		1	it		3	i		5
Thus qpA - фд - \ - 4ЯХ « 0-9 V
339
3.183 Indicate the currents in all the branches using charge conservation as shown in the figure. Applying loop rule, - А ф = 0 in the loops 1A781, 1B681 and B456B, respectively, we
get
= 0o ~ h)	(1)
l3R3 + iiR2 -	= 0	(2) and
(i'i - i3) R -	- ij R3 = 0	(3)
Solving Eqs. (1), (2) and (3), we get the sought current
(I1 ~ ‘з) ~ Я(Я2 + Я3) + r2r3
3.184 Indicate the currents in all the branches using charge conservation as shown in the figure.
Applying the loop rule (- A ф « 0) in the loops 12341 and 15781, we get
“	+ h> ” (x*i “ *3) ^2 e 0
and (iy - i3)R2-	+ h ^3 ж 0
Solving Eqs. (1) and (2), we get
=	(^2 + ^3) + ?2 R2
^1^2 + ^2^3 + ^3^1
Hence, the sought p.d.
Фа ~ Фв = ^2 “ z3^i
(1)
(2)
3.185
3.186
%2^з(^1*^2) "	* ^3)	_ * у
^1^2 + ^2^3 + ^3^1
Let us distribute the currents in the paths as shown in the figure.
Now, <Pi - <p2 “ 1	+ h &2
and «Pi - Ф3 «	+ (i-iy)R3
Simplifying Eqs. (1) and (2) we get . R3 (<P1 - Ф2) + R2 (<P1 - <Рз) Q7 A 1 ‘ R3R2 + R2R3 + R3R3
Cunent is as shown. From Kirchhoff’s Second law
*1^1 ж >
+ ( h ~ £*з ) ^2 ж >
^3 + (/3 + /2)7?4ж V
A
(1)
(2)
в
^2 R3> D г2+г3
340
Eliminating i2
h (+ ^2) ” *э^2 ж
г1^(Я3+Я4) + »3Я4-У
Hence
or,
Я,Я2
i3 Я4(Я1+Я2) + -р(Яз + Я4) л-з
я.
-V (Я1 + Я2)-^-(Яз + Я4) лз
. RtCR^R^-R^Rt+Rj)
'3"яэЯ4(Я1+Я2) + Я1Я2(Яз + Я4)
On substitution we get i3 « 1-0 A from C to D
3.187 From the symmetry of the problem, current flow is indicated, as shown in the figure.
Now, <рл - фй - i\ r + (i - ij) R	(1)
In the loop 12561, from - Д ф « 0
(i - ij R + (i - 2ij) r - r « 0
or,
(R + r). 3r + R
Equivalent resistance between the terminals A and В using (1) and (2),
_ Фл~Фв [Зг + Я__________ i r(iR + ry
K° “ i	i	3r + Я
3.188 Let, at any moment of time, charge on the plates be and -q respectively, then voltage across the capacitor, ф « q/C	(1)
Now, from charge conservation, •	•	u
i* r. + u, where b w , 1	2’	dt
In the loop 65146, using - Д ф * 0.
с + (1з+а)л’§ж0
[using (1) and (2)]
In the loop 25632, using - А ф » 0
-^ + '1Л-° or,	(4)
341
From (1) and (2), dq n 2q	dq dt
aiR-^c °’’ 5 c
On integrating the expression (5) between suitable limits, 4	1	2g
/dq 1 r ,	C, * “ C I
“ -21П“-Я ° 5” с 0
(5)
Thus
J-V.
т __е-2г/ЛС
3.189 (a) As current i is linear function of time, and at r = 0 and Ar, it equals i0 and zero respectively, it may be represented as,
• • Л и I = In 1 - — u I Af/
Ar	Ar
Jr I t\ i0&t idt = I iQ 11Jr = —— J I АГ I Z 0	0	\ f
So>	‘0=^
Hence,	i = ^-(1 -
’	Ar I Ari
The heat generated.
AT
H = f i2Rdt= f	Rdt =
J	J bt\	to]	3 Ar
о	0 L V ' J
(b) Obviously the current through the coil is given by r/Ar
I= f° И
Then charge q = J*idt = J* iQ 2	dt =
0	0
And hence, heat generated in the circuit in the time interval r [0, <»],
0
0
142
L190 The equivalent circuit may be drawn as in the figure.
Resistance of the network = Ro +
Let, us assume that e.m.f. of the cell is then current
A0 + (A/3)
Now, thermal power, generated in the circuit
P = i2 R/3 = ----------r(«/3)
(яо + (*/з))2
For P to be maximum, — « 0, which yields ui\
R = 3RQ
3.191
We assume current conservation but dissipated is
P () « ii + (i -
= i2 (Rr + R2) - 2iirR2 + i2R2
_2
not Kirchhoff’s second law. Then thermal power
;2
^1^2
+P2
• R2
11	-^1+^2
The resistances being positive we see that the power dissipated is minimum when
.	.	*2
h~lR^R2
This corresponds to usual distribution of currents over resistance joined is parallel.
3.192	Let, internal resistance of the cell be r, then V=?-ir	(1)
where i is the current in the circuit. We know that thermal power generated in the battery.
Q=?r	(2)
Putting r from (1) in (2), we obtain, Q = (£ - V) i = 0-6 W
In a battery work is done by electric forces (whose origin lies in the chemical processes going on inside the cell). The work so done is stored and used in the electric circuit outside. Its magnitude just equals the power used in the electric circuit. We can say that net power developed by the electric forces is
P = -7V = - 2 • 0W
Minus sign means that this is generated not consumed.
343
3.193	As far as motor is concerned the power delivered is dissipated and can.be represented by a load, Rq . Thus
R-^Rq	»------—।
and P~ I2R0- V R°,	к	>&>
° (Ло+А)2	f
This is maximum when Ro « R and the current	•--------------1---
/ is then
The maximum power delivered is
Y1-p
4R ““
V2	V2
The power input is ——— and its value when P is maximum is —
R + Rq	2л
The efficiency then is ~
50%
3.194	If the wire diameter decreases by d then by the information given
V2
P « Power input « — « heat lost through the surface, H.
Now, H oc (1 - 5) like the surface area and Я «(1-6)" 2
V2	9	9
So, -£-(l-d)2« A(l-d) or, V2 (1 - d) - constant
But	V « 1 + T| so (1 + т|)2 (1 - 6) = Const « 1
Thus	5 » 2 T] « 2%
3.195 The equation of heat balance is
V2	dT
Put	T-T0‘l
V2	к V2
So,	Cl + k^-^~ or, l +
d	V ^kt/c
“•	''ci'
fe л V л kt/ с , д or,	le	+ A
where A is a constant. Clearly
V2
S « 0 at t = 0, so A = - — and hence,
*7’ т	(а kt/C\
T’T^^~e >
344
3.196
Let, <Рл - <Рв ” Ф
Now, thermal power generated in the resistance R}
_________R2
*x ^2 + Rj 1^r2+rx
For P to be independent of Rx,
- 0, which yeilds ul\v
P = i'2R
2
R
. i_____R
A*—’---~
Ri
3.197
3.198
В
Al A2 12Q X *l+*2
Indicate the currents in the circuit as shown in the figure. Appying loop rule in the closed loop 12561, - Acp = 0 we get
i1 A - ^1 + iAi» 0 and in the loop 23452, (i - iy) R2 + ^2 ” h e 0 Solving (1) and (2), we get, . _	11 ^2 +
11 “ RR^+R^ + RRi So, thermal power, generated in the resistance A, 2
A
(1)
(2)
6
S/4-
11 ^2 ^^2^1
R R± + R± R2 + R R2 dP
For P to be maximum, - 0, which Helds
dR
P~ l2r
Hence,
R
R,
1
I'
Rx
4
T^2
r2
*----2---*-----3
I	2	l-u
Al R2
r~r^r;
, _ (^1r2 + i2r1)2
AR.R^R. + RJ
Let, there are x number of cells, connected in series in each of the n parallel groups N then, n x = N or, x - — n
Now, for any one of the loop, consisting of x cells and the resistor R, from loop rule
iR + —xr -x£ « 0 n
(1)
So,z' =	----
R + — n
using (!)
346
Heat generated in the resistor R,
e= i2J?= ( Nn^ ] R \n2R+NR
(2)
and for Q to be maximum,^- = 0, which yields
n -
3.199 When switch 1 is closed, maximum chaige accumulated on the capacitor, (1) and when switch 2 is closed, at any arbitrary instant of time,
q/C,
because capacitor is discharging.
<1
1
or,
(Я1+Я2)С
S"w~~
1
Ri
о
Integrating, we get
-t
i 9
Яшах" (Я1 + Л2)С °Г’ q~ 9““e
Differentiating with respect to time,
(Л1+Л2)С
(2)
i (A . 41 = q	e (Rt+/yc f----1----
w dt Чаах	I (Л1+Л2)С
or,
I(f),
(R1+RJC
Negative sign is ignored, as we are not interested in the direction of the current.
thus,	i (r) = = e ^+R2>c	(3)
+ ^2/
When the switch (Sw) is at the position 1, the charge (maximum) accumalated on the capacitor is,
4 - C*>
When the Sw is thrown to position 2, the capacitor starts discharging and as a result the electric energy stored in the capacitor totally turns into heat energy tho’ the resistors Rr and R2 (during a very long interval of time). Thus from the energy conservation, the total heat liberated thoJ the resistors.
H - U{ - ^ = lc|2
• 2C 2 ъ
346
During the process of discharging of the capacitor, the current tho’ the resistors and R2 is the same at all the moments of time, thus and H2 <* R2
a Rx
H R1 H1 = (Я, + /Q
So,
(as H
Hence
CR' _ '2 5
г . 1 __________
1	2 Rr + Л
3.200
3.201
When the plate is absent the capacity of the condenser is r eo5
C l-T)
|7 2
2(1-П)
When it is present, the capacity is c, eos d(l-T))'
(a)	The energy increment is clearly.
ДС7 - lev2-IcV2 2	2
(b)	The charge on the plate is CV
q-~ -—— initially and q?- CV finally.
CVn	CV2T)
A charge у—has flown through the battery charging it and withdrawing ——units of energy from the system into the battery. The energy of the capacitor has decreased by 1 CV2r\
just half of this. The remaining half i.e. — j--- -1 must be the work done by the external agent in withdrawing the plate. This ensures conservation of energy.
Initially, capacitance of the system = C e. 1	2
So, initial energy of the system : Ui» — (С e) V
1	2
and finally, energy of the capacitor :	— С V
Hence capacitance eneigy increment,
ДС7- |cV2-^(Ce) V2- CV2 (e - 1)- -0-5 mJ
From energy conservation
A “ ^cdl + ^agent (as there is no heat liberation) But A^, = (C; - Vs = (C - Cz)V2'
347
Hence Agent “ Д 17 "Adi
- |c(l-e) V2= 0-5mJ
3.202 If Co is the initial capacitance of the condenser before water rises in it then
1 о	en 2/л R
Ui - | C0V2 , where Co -
(R is the mean radius and I is the length of the capacitor plates.)
Suppose the liquid rises to a height h in it. Then the capacitance of the condenser is
a	a	a
and energy of the capacitor and the liquid (including both gravitational and electrosatic contributions) is
i е02лЛ	~	л
4	(/ + (e-l)A)V2 + pg(2nRhd) J
2 a	2
If the capacitor were not connected to a battery this eneigy would have to be minimized. But the capacitor is connected to the battery and, in effect, the potential energy of the whole system has to be minimized. Suppose we increase h by 5Л. Then the energy of the capacitor and the liquid increases by
bh 1-^- (e-1)V2 + pg(2xRd)h\
and that of the cell diminishes by the quantity which is the product of charge flown and V
., eo (2лЛ) о
bh-------— (e - 1) V
a
In equilibrium, the two must balance; so e0(e-l)V2 2d
pgdh
Hence
h =
e0(e-l)V2 2pgd2
3.203 (a) Let us mentally islolate a thin spherical layer with inner and outer radii r and r + dr respectively. Lines of current at all the points of this layer are perpendicular to it and therefore such a layer can be treated as a spherical conductor of thickness dr
2
and cross sectional area 4 л r . Now, we know that resistance,
Integrating expression (1) between die limits,
348
or,
1 1’
a b
Capacitance of the network,C «
4 л e0 e
1 Г’ a b
and
C qp
’ where q is the charge ' at any arbitrary moment
also,	qp =	as capacitor is discharging.
From Eqs. (2), (3), (4) and (5) we get,
(2)
(3)
(4)
(5)
<1-
dt
q pee0

Integrating
qQe p£o6
Hence
(b) From energy conservation heat generated, during the spreading of the charge,
Я = 17, - Uf [because » 0]
2	2
1 #o 11 n ,4o b -a ----------- — — — —. (j » .......... .....
2 4 я eoe a b 8 л e0£ ab
3.204 (a) Let, at any moment of time, charge on the plates be (qQ - q) then current through
the resistor, i = ----j------, because the capacitor is discharging.
at
or,	dt	-(Чо-ч.) |	
Now, applying loop rule in the circuit,			
	0	c	
or,	dt	C	R	
or,	—l-dt q0-q RC	i-d<L dt	
349
3.205
At Г» 0, q = 0 and at t = t, g = q
So,
, %-? -T
In----= 7Г77
%
q = q0(l-e~x/RC}- 0-18 mC
(b) Amount of heat generated = decrement in capacitance energy 2
.	. I	-e ~X/RC и
Thus
2C 2
2 г	2т
|^-[1-е‘лс]- 82mJ £ tz L	J
Let, at any moment of time, charge flown be q then current i=
Applying loop rule in the circuit, - Acp - 0, we get :
J# TD Vo ~ fl) _2_ _ Л c + c~
dt
or,
dq 1	,
CVQ-2q~ RC
So, In
cv0
or,
CV I — q~ -"2^(1 ~eRC
- 2 for 0 s t s t RC
~(CVo-^)
-c cvb-q,
i=di dt
C
R
Hence,
. _ dq __ C Fo 2 l“ ~dt~ ~2~RC
Now, heat liberaled,
-2t/RC Vo 21/ЯС
e “ Re
Г	v2 r
Q = | i2Rdt = -^R I e
J	R2 J
о	0
RC dt=±CV2
3.206
In a rotating frame, to first order in co, the main effect is a coriolis force 2 m v* x ox This unbalanced force will cause electrons to react by setting up a magnetic field В so that the magnetic force e v x В balances the coriolis force.
e -z*	2m ->
Thus	- т— В = co or, В = - — co
2m	e
The flux associated with this is
Ф= Nnr2B= Nnr2 — to e
350
where N =  ----is the number of turns of the ring. К (0 changes (and there is time for
2 л г
the electron to rearrange) then В also changes and so does Ф. An emf will be induced and a current will flow. This is
I- Nnf — a/R
e
The total charge flowing through the ballastic galvanometer, as the ring is stopped, is
q = Nnr2 / ^~w/R 1 e
_	e	Iwr
S°’	W
3.207	Let, n0 be the total number of electoms then, total momentum of electoms, P~ nomevd	(1)
Now,	I- pSxvd- ~^Sxvd. ^-vd	(2)
Here Sx = Cross sectional area, p = electron charge density, V = volume of sample
From (1) and (2)
p-	0-40(1 Ns
3.208	By definition
n e vd = j (where vd is the drift velocity, n is number density of electrons.)
I nel
Then	т - — = —7~
*d J
So distance actually travelled
(<v> = mean velocity of thermal motion of an electron)
3.209	Let, n be the volume density of electrons, then from I - p Sx v#
I- | < v> | « neSxj
neSl
So,	r»—y^-3ps.
(b) Sum of electric forces
= | (nv) eE| = \ nS lepjX where p is resistivity of the material.
= nS/ep^-» nelpl» 1*0 pN о
351
ЗЛЮ
From Gauss theorem field strength at a surface of a cylindrical shape equals, 2 ^e~ w^erc k linear chaige density.
Now, Also, or,
eV	or,	-/2е V v= Д/ V me
dq • к dx so,	dq 5 dx dt" K dt
г л	. I	I
I - AV or, A= = -у		’ using (1)
	/2ёТ
	V in
(1)
Hence	£~ t-2— V	“ 32V/m
2 л SqT 2eV
(b) For the point, inside the solid charged cylinder, applying Gauss’ theorem, 2ягЛ£- xr2h--------------------------------------<
е0я7?21
or,	E « —zr - ---------------у
2jie0jRz 2яе0/?2
So,from	£-
dr
*2
R
<₽t
------zrdr 2яе0£2
or,
Ф1-Ф2
X
2 ле0Л2
о" 4яе0
Hence,
2
Ф1 ” Ф2 “
0
3.211
4/3
Between the plates cp - a x
dq>	4 1/3
flx-x
dx	3
tAp 4	-2/3	/
^9^	“_p/e°
or,
ft 4eoe -2^
P-----9-x
Let the chaige on the electron be - e,
352
1	2
then	2mV ” e = Const = 0’
as the electron is initially emitted with neligible energy.
2	2 e <p - /2 ecp
v = -----x v== л/-------n
m v m
c	eo - /2 <p -2/3
So,	j = - p v= —-—V —212 x
’	J H 9 v m
(j is measued from the anode to cathode, so the - ve sign.)
V
3.212 E = -a
So by the definition of the mobility +	+ V _	- V
V « u0 d, V « MO“J
+	eV
and	j- (»_ u0 + n_ u0)
(The negative ions move towards the anode and the positive ion towards the cathode and the total cunent is the sum of the currents due to them.)
On the other hand, in equilibrium n+ = n_
_	I / . + ..eV
So,	n+ = n_ = -/ (u0 + u0) —
3.213
=-------—-------2-3xlO8cm-3
e VS(u0 + u0)
Velocity = mobility x field
Vo
or, v = и — sin co t, which is positive for 0 £ co t & л
So, maximum displacement in one direction is
/Vo	2«V(
u— sincotdt -
0
2«V0
At co = co0, /, so, —--------I
ml2
Thus	и -
3.214 When the current is saturated, all the ions, produced, reach the plate.
Then,	Й- -	- 6 x IO9 cm-3 s-1
1 eV
(Both positive ions and negative ions are counted here) . x.. ,	. dn	2
The equation of balance is, -^ =	- rn
353
The first term on the right is the production rate and the second term is the recombination rate which by the usual statistical arguments is proportional to иI 2 (= no of positive ions x no. of -ve ion). In equilibrium,
so,
n
6 x 107 cm"3
3.215	Initially n  n0 - Vwx- /r
Since we can assume that the long exposure to the ionizer has caused equilibrium to be set up. Afer the ionizer is switched off,
or	rdt- or, rt — — + constant
л2	n
But	n - nn at t - 0, so, rt -----
u	* ”o
The concentration will decrease by a factor t) when ,	1	1 n-i
Г tg “ -/-------- ----
Vn no no
Л -1 or,	tQ- 4==.- = 13 ms
Vr и;
3.216	Ions produced will cause charge to decay. Clearly, CqA T) CV « decrease of charge «	eA dt • -j- V T)
e0 Vt) or,	r- -----л - 4-6 days
nied2
Note, that ni9 here, is the number of ion pairs produced.
3.217	If v « number of electrons moving to the anode at distance x, then dv	ax
— • av or v - vne dx	°
Assuming saturation, /= evoea<*
3.218 Since the electrons are produced uniformly through the volume, the total current attaining saturation is clearly,
d
I» Г e (n-A dx) eax « en-Al-------]
J	I	a I
о	'	'
I . fead-l\ Thus,	j= enA -------------
A 4 a I
354
3.5 CONSTANT MAGNETIC FIELD. MAGNETICS
3.219 (a) From the Biot - Savart law,
So, IT- db<R+f dl**?
Since x is a constant vector and | R | is also constant
and
dl^R-f Rdln"
- dl* 2 л Я 2 л*
Here n is a unit vector perpendicular to the plane containing the cunent loop (Fig.) and in the direction of x
„	Б* Ho 2n/f2i -
Thus we get	В *  ------т,--
6	4л tf+Jt*)372
2 л
3.220 As LAOB = —, ОС or perpendicular distance of any segment from centre equals n
R cos —. Now magnetic induction at O, due to the right current carrying element AB
H0 I . n ----------2 sin —
4л o л n
A cos — n
(From Biot- Savart’s law, the magnetic field at О due to any section such as AB is perpendicular to the plane of the figure and has the magnitude.)
355
,	C.. ; jRcos—sec26d0	•	.
Ho . dr n j Но1 n	пН(/1_.л
— i “vcosO - I ~----------cosO ------2sm—
4л r* J 4л „2 2я 2Л 4л _ л п ^ R cos—sec 0	Bcos—
_*	п	п
As there are n number of sides and magnetic induction vectors, due to each side at O, are equal in magnitude and direction. So, D Но ш’ . я Bn = ---------------------2 sin — -n
° 4л „ я R cos — n
Но т л ------tan — n
and for n -> oo
2 л R
В - —i Lt °	2 R
tan — ____n w
dv
Ho £ 2 R
3.221 We know that magnetic induction due to a straight current carrying wire at any point, at a perpendicular distance from it is given by :
В *	- (sin 0. + sin 02 ),
4 л r 1	2
where r is the perpendicular distance of the wire from the point, considered, and 0X is the angle between the line, joining the upper point of straight wire to the considered point and the perpendicular drawn to the wire and 02 that from the lower point of the straight wire.
Here,
л « Ho i Г Ф Ф
R = B^ = ~----------------J cos + cos x
1 J 4 л >	. ф |	2	2
(d/2) sm I
and
»	» Ho i ( . Ф
B9 = Вл = --------------sin * + sm
(d/2) cos *• \
Hence, the magnitude of total magnetic induction at O,
Bo« Вг + В2 + В3 + В<
Ho 4i 4 л d/2
Ф . Ф cos -J- sm
2 ______2.
sin cos
4Ho*
——- 010 mT я a sin ф
356
3.222 Magnetic induction due to the arc segment at O,
Bm- 72-|(27t-2qp) arc 4 л Я
and magnetic induction due to the line segment
at O,
Д;п =	----[2sin(p]
line 4 л R cos ф 1 VJ
So,	total magnetic induction at O, Hn i
Bo= B„c + Bhnc- —r [л - <p + tan <p] = 28цТ
3.223
(a)	From the Biot-Savart law,
dB~
Ho . (dl x 4л1 r3
So, magnetic field induction due to the segment 1 at O,
(b)	Here, B, -	, B, - 0,
v '	1 4 л a a 1
—	M-0	1	лее
B^ » -— — sm 45 , 5	4 л b
D	HO l • A CO
Ba* sin 45°, 4	4 л b
and	B5» 0
So, Bq ж By + B2 + B3 + B4 + B5
Ho 1 3 л л Ho 1 . a_q Ho 1 . A_o A
»-----— + 0 + -— — sm 45° + -— — sm 45° + 0
4л a 2 4л о	4лЬ
.М2_.ГЗл V2_ 4xl 2a + b
357
3.224 The thin walled tube with a longitudinal slit can be considered equivalent to a full tube and a strip carrying the same current density in the opposite direction. Inside the tube, the former does not contribute so the total magnetic field is simply that due to the strip. It is ш Ho (I/2xR)h = h)//» “ 2л r “ 4л2Лг
where r is the distance of the field point from the strip.
—►
3.225 First of all let us find out the direction of vector В at point O. For this purpose, we divide
the entire conductor into elementary fragments with current di It is obvious that the sum of any two symmetric fragments gives a resultant along 2Гshown in the figure and con-—>
sequently, vector В will also be directed as shown
So,
Ho j. .
——-di sinqp 2jiR y
Г Ho . .	, (	..	i‘ .
I —2—tsmcpacp, as Л- —d<p
J 2 лх R	I
о	x
2
Hence	цог/л R
3.226 (a) From symmetry
« Br+B2+ B3
(b) From symmetry ^o “	+ ^2 + &3
Ho i Ho i Зл л Ho i L 3 л' «	+ т—~ — + 0 « -—~ 1 +
4 л Я 2л Я 2	4 л Я 2
(с) From symmetry
^о ж + ^2 +
, *L.L + h-i. n+*LL- ±2-i(2 + J
4лЯ 4лЯ 4лЯ 4л/Г
3.227 Во-В1 + В2
—>	Hn i /—
or, |В0|=	- 20 цТ, (using 3.221)
358
3.228 (a)
Bo« Br+B2+B3
- ±Ll(_f) + ±Ll
4лЛ' ’ 4лЛ
Ho i 4л R
So,
Mo i г - 7* T*
+ 4 - 0-30 j»T 4 л R
(b)
Бо« +	+
Ho i z г*ч Ho i
= 7— 77 (- * ) + 7— 77
4лR 4nR
Ho i 4nR
So,
|Во|» ^£V1 + Ot + 1)2- 0-34цТ
(с) Here using the law of parallel resistances
•	•	• л *1	1
*2	3
So,
h + h 4
h ‘3
„	3 .	. .	1 .
Hence	и - ~ i, and t. • — i
£ 4	1 4
_„	•=♦ Ho i t 7*4 Ho i , r*4
1Ъи +
±в./3я\»1г T* Mo (^/2)^^ 4л^ 2 jjR1-1 } + 4л R 1
Thus,
-Mo i 4л R
(T+k)+O
011 “T
3.229 (a) We apply circulation theorem as shown. The current is vertically upwards in the plane
and the magnetic field is horizontal and parallel to the plane.
f в’-dT- 2Bl~ i^il or,
(b) Each plane contributes ц0 ~ between the
planes and outside the plane that cancel.
Thus
piot between the plane 0 outside.
359
3.230	We assume that the current flows perpendicular to the plane of the paper, by circulation theorem, 2Bdl- p0(2xd/)j
or,	B- p^xj, |x|sd
Outside, 2 В dl- \iQ(2ddl)j
or,	В - p0 d j | x| st d.
3.231	It is easy to convince oneself that both in the regions. 1 and 2, there can only be a circuital magnetic field (i.e. the component B^). Any radial field in region 1 or any Bz away from the current plane will imply a violation of Gauss’ law of magnetostatics, Вф must obviously be symmetrical about the straight wire. Then in 1,
Вф2лг- Ио/
or,
D Ho I
------
ф 2 я г
In 2, #ф • 2 я г - 0, or Вф « О
3.232	On the axis,# - ----2- В* along the axis.
2 (R + x )
Thus,
00
J* В • dr*** J* ВXdx-— 00
C dx
2 J (R2 + x2)3/2
- 00
x/2
^ir2 f T?sec20dO 2 J R 3 sec3 0
-x/2
on putting x - R tan 0
x/2
- J cos0J0-
-x/2
The physical interpretation of this result is that J Bxdx can be thought of as the circulation — 00
of В over a closed loop by imaging that the two ends of the axis are connected, by a line at infinity (e.g. a semicircle of infinite radius).
3.233 By circulation theorem inside the conductor НоЛяг2 ог> РоЛг/2
-» 1 г» —
i.e.,	В--Ио/хг
Similarly outside the conductor,
360
i 1 я2 Вф2лг-	or« B<t~ 2^г~
с	Б* 1
So,	5--ц0(;хг) —
2.234 We can think of the given current which will be assumed uniform, as arising due io a negative	^x***~'*\
cunent, flowing in the cavity, superimposed on the true current, everywhere including the / cavity. Then from the	previous problem, by	/
superposition.	I	\	I
\	A	J
B- -poj*(Ap-Bp)- -^jxl	\	J
If I vanishes so that the cavity is concentric with the conductor, there is no magnetic field in the cavity.
3.235 By Circulation theorem, r
ц0 j* j (rf) x 2 л r' dr' о
or using Bv • brainside the stream, r bra+l- Ho J j(r')r'Jr' 0
So by differentiation, (a + l)Z>r“- Hoj(r)r
,r	• i , b(a+ 1) a-i
Hence,	j (r) « ------- r
Ho
3.236	On the surface of the solenoid there is a surface current density
A
Then,	В » - nl f Rd<pdz 3-"
4л J	r03
where is the vector from the current element to the field point, which is the centre of the solenoid, Now,	-ефх^ж Rez
r0-tf+R2)v2
Thus,
-//2
361
(on putting z = Я tan a)
p0 и/sin a =
И0И/ V(Z/2)2 + Л2
3.237	We proceed exactly as in the previous problem. Then (a) the magnetic induction on the axis at a distance x from one end is clearly,
ю Ип	2 I dz	1 r n2 I dz
B= ——х2лЯ I —z--------------7-477= -цои/А I —7-------7477
4jl	J [A 2 + (z - x)2 ]3Z2 2го	J (z2 + R2)3/2
0	x
k/2
= \\kQnI f cos ed6 - ~Ho1 - T I Vx2+A2
tan Л
x > 0 menas that the field point is outside the solenoid. В then falls with x. x < 0 means that the field point gets more and more inside the solenoid. В then increases with (x) and eventually becomes constant, equal to ц0 n I. The В - x graph is as given in the answer
script.
(b)
Bn-f>B
We have, —------
^0
.  °- - - l-2r] Va2+x02
- 1-n
or,
Since T| is small (- 1%), x0 must be negative. Thus x0 « - | x01
and
or,
lXol . .
.	=» 1-21]
Va2 + |x0 I2
|x0|2- (1-4т) + 4т}2) (A2 + |x0|2)
0- (1 - 2tj)2A2 - 4r| (1-t)) |x0|2
,	<1-2П)А.
2VW^)
3.238	If the strip is tightly wound, it must have a pitch of Л. This means that the current will flow obliquely, partly along еф and partly along e? Obviously, the surface current density is,
7*- -[Vl -(Л/2лА)2 e + e .
362
By comparision with the case of a solenoid and a hollow straight conductor, we see that field inside the сой
= Ио£У1-(Л/2лЯ)2
(Cf.B- nonZ).
Outside, only the other term contributes, so
Бфх2№ Н0р^х2^
or,
3.239
3.240
я -4л г *
Note - Surface current density is defined as current flowing normally across a unit length over a surface.
Suppose a is the radius of cross section of the core. The winding has a pitch 2лЯ/У, so the surface current density is
J* " 2stR/N 61 + 2лае2 where e^ is a unit vector along the cross section of the core and e2 is a unit vector along its length.
The magnetic field inside the cross section of the core is due to first term above, and is given by
[i0NI
(NI is total current due to the above surface current (first term.))
Thus,	- n0NI/2xR.
The magnetic field at the centre of the core can be obtained from the basic formula.
Но Л x К
------5—dS and is due to the second term.
4я 'о
dB~
So,
or,
В =	' ~~~ | "^7 Rdy x 2na
z z z 4 л 2na Jr3
b- —
z 2R
N
The ratio of the two magnetic field, is = — л
We need the flux through the shaded area.
Now by Ampere’s theorem;
or,
t 2
B„ 2nr = un —о • nr ф °лЯ2
The flux through the shaded region is,
363
л
Ф1 “ J 0
О
л
I ar—J—j =
J 2я
4л
3.241
Using 3.237, the magnetic field is given by,
B- |pion/71--	.
20
3.242
At the end,B « i p0 nl = ^Bo, where BQ « p0 nl,
is the field deep inside the solenoid. Thus,
Ф = pt0 nIS = Ф0/2, where Ф = [iQnIS
is the flux of the vector В through the cross section deep inside the solenid.
Bv2itr- HgNI
2лг
or,
ъ
Then,
/Ho
Brn hdr. a ss r ss b = -— 2N th Inn, where ri = b/a ф ’	4л	h 1
3.243
3.244
Magnetic moment of a current loop is given by pm - n i S (where n is the number of
turns and S, the cross sectional area.) In our problem, w=l, 5=лЛ2 and В =
2BR _2 2лВЯ3 p = ------лА = ---------
Ho	Ho
N
Take an element of length rd 0 containing — rd® turns. Its magnetic moment is лг
-de-^d2! л 4
normal to the plane of cross section. We resolve it along OA OA integrates to
X
So,
and OB. The moment along
I ^d2IdQcasQ- О
J 4 о while that along OB gives
Л d I • r\ J(X 1 ж r I 2 r p= I —a— sm 0 dQ = ~Nd I m J 4	2
A
0
0
364
3.245 (a) From Biot-Savart’s law, the magnetic induction due to a circular current carrying wire loop at its centre is given by,
The plane spiral is made up of concentric circular loops, having different radii, varying from a to b. Therefore, the total magnetic induction at the centre,
(i)
Ho
where — i is the contribution of one turn of radius r and dN is the number of turns in 2r
the interval (r, r + dr)
N
i.e.	dN = -------dr
b-a
Substituting in equation (1) and integrating the result over r between a and b, we obtain, b
/Ho’ N , P-qIN b
-r— Ti----г dr = 77-7:---г In —
2r (b - a) 2 (b-a) a
(b) The magnetic moment of a turn of radius r is pm = in r2 and of all turns, b
C м f 2 N , niN(b3-a2) p = I pmdN» I in r   dr = ——; ~~L r J	J b-a 3 (b - a)
3.246 (a) Let us take a ring element of radius r and thickness dr, then charge on the ring element., dq = о 2 nr dr
.	4. j +	1	+ (a 2 л rdr) co	,
and current, due to this element, ai - ----------— » a co r dr
2n
Ho di
So, magnetic induction at the centre, due to this element : dB =
R
r Г ц0 о cor dr p0 and hence, from symmetry : В - J dB - I ------------ — а соЯ
о
(b) Magnetic moment of the element, considered, dpm = (di) яг2 = a co dr n r2 = a л co r3 dr
Hence, the sought magnetic moment,
R c , C 3i	B4
Pm=J dPm^ J °™Г	= ОСОЛ —
0
365
3.247 As only the outer surface of the sphere is charged, consider the element as a ring, as shown in the figure.
The equivalent current due to the ring element,
di- — (2 лг sin 0 rd 0) о	(1)
and magnetic induction due to this loop element at the centre of the sphere, O,
Ио 2 я r sin 0 r sin 0 Но sin2 0 Jo- — di-----------=----------- —di------
4л	4л r
[Using 3.219 (b) ]
Hence, the total magnetic induction due to the sphere at the centre, O, л/2
„ c j Ho co 2лг28ш0</0 8т20о r .
5 “	" J	2^----------r---------[USmg (1)]
0
л/2
Hence,
J Но о cor a	?
——— sin3 0 d 0 • — p0 a cor » 29 pT
о
3.248
The magnetic moment must clearly be along the axis of rotation. Consider a volume element dV. It contains a charge-®—r dV, The rotation of the sphere causes this charge
4л/ЗЯ3
to revolve around the axis and constitute a current.
За rrr
—*-;dVx —
4nR3 2л
Its magnetic moment will be
—^-rdVx ~-x лг2 sin20 2л
So the total magnetic moment is
R л
Г Г Зя 2 . cor2sin20, 3q co R5 4 1 D2 p - I I —-^rsinOJOx------------Jr-—-*7 x — x — x — - -rqR co
m J J 2R3	2	2Я3 2	5	3	5 *
о 0
The mechanical moment is
M- |mfl2co, So, ?7- A 5	M 2m
3.249 Because of polarization a space charge is present within the cylinder. It’s density is p- -div P- -2a
Since the cylinder as a whole is neutral a surface charge density ap must be present on the surface of the cylinder also. This has the magnitude (algebraically)
366
cr х 2л/? = 2 а л/?2 or, а = а/? р	9 р
When the cylinder rotates, currents are set up which give rise to magnetic fields. The contribution of pp and op can be calculated separately and then added.
For the surface charge the current is (for a particular element) CO 2 aR x 2nRdx x — - aR wcbc 2л
Its contribution to the magnetic field at the centre is p0/?2 (a/?2codr) 2(x2 + R2)3/2 and the total magnetic field is - 00	00
/ц0/?2 (a/?2 codr) p0a/?4co C fa p0a/?4co 2	2
2(J+R2)3/2	2“J (x2 + /?2)3/2	2
00	- 00
As for the volume charge density consider a circle of radius r, radial thickness dr and length dx.
The current is- 2а x 2 л r dr dx x « - 2 ar dr co dx 2л
The total magnetic field due to the volume charge distribution is R »	2	R	«
Bv = - I	dr I	dx 2 л r co-Лтгл ж	“ I	а Ho 00 г* dr I	(x2 + r2)3/2
v J J	2(x2 + r2)3/2	J
0-OO	v	'	0	- 00
R
« -J* а p0 cor dr x 2 ~ - ц0 a co R 2 so, В « Bs + Bv = 0 0
—>	>
3.250 Force of magnetic interaction,/*^ « e (у x В)
Where.	B-
4л r3
So,	^7[Гх(Гх^]
Ho e2 r/	—» , > > —►, Ho e2 , 2 —*>
= 4^7[(?Г)ХУ-(1"’Г|ХГ]= 4^?(‘V П
Hence,
ijL,j I Fdectic I
- v2H0 So-
l-00 X 10” 6
367
3.251 (a) The magnetic field at О is only due to the 	>	j
curved path, as for the line element, d I f f r.
-* i	—>	i ->
Hence,
—»	—»	iu i2	—*
ThusFu- iB(-j)»	)
So,Fu =	0'20 N/m
4л
(b) In this part, magnetic induction В at О will be effective only due to the two semi infinite segments of wire. Hence
~ Ho1 • n
В = 2-----7~j~\ sm у (~ *)
’ 7Г<-Г>
Thus force per unit length,
3.252	Each element of length dl experiences a force BI dl. This causes a tension T in the wire. For equilibrium,
This must equals the breaking stress am for rupture. Thus,
4IR
3.253	The Ampere forces on the sides OP and O' P are directed along the same line, in opposite directions and have equal values, hence the net force as well as the net torque of these forces about the axis 00’ is zero. The Ampere-force on the segment PP' and the corresponding moment of this force about the axis OO' is effective and is deflecting in nature.
368
In equilibrium (in the dotted position) the deflecting torque must be equal to the restoring torque, developed due to the weight of the shape.
Let, the length of each side be I and p be the density of the material then,
UB (I cos 0) = (S I p) g ~ sin 0 + (S I p) g sin 0
+ (S I p) gl sin 0
or, il2В cos 0 « 2 S p gl2 sin 0
Hence,	В - tan 0
i
3.254	We know that the torque acting on a magnetic dipole.
But, p?m  iS n, where n is the normal on the plane of the loop and is directed in the direction of advancement of a right handed screw, if we rotate the screw in the sense of current in the loop.
On passing a cunent through the coil, this torque acting on the magnetic dipol, is counterbalanced by the moment of additional weight, about O. Hence, the direction of current in the loop must be in the direction, shown in the figure.
_—>	—»
pmxB~ - lx tong
or, NiSB- &mgl
So,
tongl NiS
0-4 T on putting the values.
B-
3.255	(a) As is clear from the condition, Ampere’s forces on the sides (2) and (4) are equal in magnitude but opposite in direction. Hence the net effective force on the frame is the resultant of the forces, experienced by the sides (1) and (3).
Now, the Ampere force on (1), r Но «о F1’2^7 n
and that on (3), „ Ио ‘о* 2^7 К
So, the resultant force on the frame
* FY - F3, (as they are opposite in nature.)
369
2 Но
----------------0-40 nN.
(b) Work done in turning the frame through some angle, A * Ji dФ = i (Фу - Ф;), where Фу is the flux through the frame in final position, and Ф-, that in the the initial position. Here,
| Фу| « | Ф,| - Ф and Ф; - - Фу ДФ « 2 Ф and А - i 2 Ф
so,
Hence,
= 2i
А» 2i I BdS
a
Ио fo a , Ио uq a , / 2n + 1 -----ar« ----InkH——
2л г я 12т| -1
3.256 There are excess surface charges on each wire (irrespective of whether the current is
——>
flowing through them or not). Hence in addition to the magnetic force Fm, we must take into account the electric force Fe Suppose that an excess charge X corresponds to a unit length of the wire, then electric force exerted per unit length of the wire by other wire can be found with the help of Gauss’s theorem.
F- -KE-
c	4 я e0 I 4 я e0/
(1)
where I is the distance between the axes of the wires. The magnetic force acting per unit length of the wire can be found with the help —>
of the theorem on circulation of vector В
F ” 4л Г
where i is the current in the wire. (2) Now, from the relation,
X « C <p, where C is the capacitance of the wires per unit lengths and is given in problem 3.108 and qp = iR
Л 7teo • „ i Inrj X= ------iR or, — » --------4-
In т|	к	ле0Я
Dividing (2) by (1) and then substuting the value of — from (3), we get, К
HO (Ini])2 Ft" eo 1?R2
The resultant force of interaction vanishes when this ratio equals unity. This is possible when R « Rfy where
(3)
370
3.257 Use 3.225
Ro =	0-36 kQ
£o л
The magnetic field due to the conductor with semicircular cross section is
В =
7l2R
Then
3F nr H0/2 _f e Dl =* n
л2 Я
3.258 We know that Ampere’s force per unit length on a wire element in a magnetic field is given by.
dFn = i (n x В ) where n is the unit vector along the direction of current.	(1)
Now, let us take an element of the conductor i2, as shown in the figure. This wire element is in the magnetic field, produced by the current which is directed normally into the sheet of the paper and its magnitude is given by,
<2>
From Eqs. (1) and (2)
I2 A
dFn = -drfnxB), (because the current through the
4 element equals — dr
—>	Un A A dr	a _ —>
So,	dF= -------------, towards left (as n 1 В ).
n 2л b r	v
Hence the magnetic force on the conductor : a + b
F = 7^- I" — (towards left) » In a + (towards left).
" 2л b J r v	' 2л b a v
a
Then according to the Newton’s third law the magnitude of sought magnetic interaction force
Ио Л Л * a + b
=	—7—In-------
2л b a
3.259	By the circulation theorem В = ц0 i,
where i = current per unit length flowing along the plane perpendicular to the paper. Currents flow in the opposite sense in the two planes and produce the given field В by superposition.
The field due to one of the plates is just The force on the plate is,
^B x i x Length x Breadth
-— per unit area. 2p0 F
(Recall the formula F = BIl on a straight wire)
371
+ B2
3.260	(a) The external field must be —-—, which when superposed with the internal field
1*1
2
(of opposite sign on the two sides of the plate) must give actual field. Now
“ ^2	1
------2^1
or,
Thus,
~^2
Ho
2|i0
Bi
B2
By — B2	Py +B2
(b)	Here, the external field must be —-— upward with an internal field, —-—.
on the left and downward on the right Thus,
. B1+B2	B?-B22
i = ----- and F = —--------.
Ho	2Mo
(c)	Our boundary condition following from Gauss’ law is, By cos Gx = B2 cos G2.
Also,^ sin Gt + B2 sin G2) = p0 i where i = current per unit length.
-, upward
The external field parallel to the plate must be
By sin Gt - B2 sin G2
2
(The perpendicular component By cos 0p does not matter since the corresponding force is tangential)
By2 sin2 G, - B2 sin2 G2
Thus, F = -----------------------per unit area
2n0
1*1 ~1*22 « —--------per unit area.
The direction of the current in the plane conductor is perpendicular to the paper and beyond the drawing.
3.261	The Current density is where L is the length
of the section. The difference in pressure produced must be,
Ap = x В x (abL)/ab -
372
3.262	Let t - thickness of the wall of the cylinder. Then,
J = 1/2 nRt along z axis. The magnetic field due to this at a distance r
Hp/2 f 8я2А3Г2 J _ t
_2p3, Л A t
Ho^2	,
e?P7 [я'+0(')1-
8 л2 Л 2
3.263	When self-forces are involved, a typical factor of — comes into play. For example, the force on a current carrying straight wire in a magnetic induction В is BIl. If the magnetic induction В is due to the current itself then the force can be written as,
I
F~ fB(r)dTl о
If В (Г ) а Г, then this becomes, F -	(I) 11.
In the present case, В (I) « p0 n I an<* this acts on nl ampere turns per unit lehgth, so, F 1 ZxnZxlx/ 1	2 r2
pressure^ = Area” 2^"—TO--------------2И°" 1
3.264	The magnetic induction В in the solenoid is given by В « p0 nl. The force on an element dl of the current carrying conductor is,
dF-^nlldl-±v^42dl £ £
This is radially outwards. The factor -• is explained above.
373
To relate dF to the tensile strength Flim we
proceed as follows. Consider the equilibrium
of the element dl. The longitudinal forces F have a radial component equal to,
dF- 2Fsiny- FdQ 1	7
Thus using Rdti, F = — R
This equals Flim when, /» /lim «
Note that Flim, here, is actually a force and not a stress.
3.265
Resistance of the liquid between the plates®
5
Voltage between the plates = Ed = v Bd,
Current through the plates -
vBd
Power, generated, in the external resistance R,
v2B2d2R v2B2d2	v2B2d2
v „ pD D vBSd
This is maximum when R « and Pmav « —-----
5 max 4p
3.266 The electrons in the conductor are drifting with a speed of,
J I
v —ж -------—
ne nR2ne
where e = magnitude of the charge on the electron, n = concentration of the conduction electoms.
The magnetic field inside the conductor due to this current is given by,
9 I	Ho Ir
B^r>nr ТТ2И0 or, 71 К	л
A radial electric field vB^ must come into being in equilibrium. Its P.D. is,
374
3.267 Here.v^ = — and j = ne vd 200x104j^x1T
SO,	П=	-------tV/to.
eE 1.6х10"1’Сх5х1(Г4
= 2-5 x 1028 per m3 - 2-5 x 1022 per c.c.
Atomic weight of Na being 23 and its density « 1, molar volume is 23 c.c. Thus number ..	. 6 x 1023	22
of atoms per unit volume is ——— = 2-6 x 10 per c.c.
Thus there is almost one conduction electron per atom.'
3.268
3.269
л ...	,	dirft velocity	v
By definition, mobility =	* ... „ ------------—г -7— or ц = —
Electric field component causing this drift	EL
On other hand, £ 1 £ = vB - —, as given so, p - — » 3-2 x IO"3 m2/(V • s) T)	y\B
Цо/
Due to the straight conductor,
We use the formula,	F = (p^- V) В
(a)	The vector /Г is parallel to the straight conductor.
because neither the direction nor the magnitude of В depends on z
(b)	The vector pm is oriented along the radius vector r
F= pm—B m dr
The direction of В at r + dr is parallel to the direction at r. Thus only the qp component
—+
of F will survive.
d	VO1 Pm
(c)	The vector p^ coincides in direction with the magnetic field, produced by the conductor carrying current I
7? d М-»
F = p_ ——-— e„ =
Гт rdtp 2л <fl
So,
Ц01р„^
F = ----z—As,
2лг2 r
2 лг2 Эф
de* d<₽
- e,
3"76
376
Неге, В' « В cos 9, Н'. - — В sin 0, В' - и В sin 0, Н' = —— cos 0 "	'Но	'	" НН0
т В cos 0 Л 1\	. т ц- 1 „ . о
J„ = ------ 1 - — I and J.« t--В sm 0 .
Mo И)	Mo
Only Jn contributes the surface integral and
£ 7* ,r* C 7» r» £ T nR2BcosQ(. 1\
-O’ JdS=-Q JdS=O JndS= ----------------------1- —
lower	lower
(b) £ B’dr^ (Bt-B't)l= (l-n)B/sin0 Г
3.275
Inside the cylindrical wire there is an external current of density —y. This gives a magnetic лД
field Hv with
2
Н2лг-1-^ or, Яю----------
’ R2 v 2л R2
|A|Aq Zr	li - 1 Zr у Ir
From this fi = ----у and 7 =	----у = ~—у = Magnetization.
” 2nR2 ” 2л r2 2лВ2
Hence total volume molecular current is,
/J dr^ I ~^—dl = vl v J 2лЯ Л
r- R
The surface current is obtained by using the equivalence of the surface current density to
J x nt this gives rise to a surface current density in the z-direction of -2лл
The total molecular surface current is,
7> -^-(2лЯ) = -x7. ZJuC
The two currents have opposite signs.
3.276 We can obtain the form of the curves, required here, by qualitative arguments.
From	H-dl = I,
we get Then	H(x»0)- H(x«0)~ nl В (x » 0) - цц0 nl В (x < 0) = ц0 nl
Also,	B(x<0)= ц0Н(х<0) J(x<0)- 0
В is continuous at x = 0, H is not These give the required curves as shown in the answersheet.
377
З.ТТ1 The lines of the В as well as H field are circles around the wire. Thus
Hr тег + H2 тег =
Also pi0 =
Thus	=
я2 =
and	В =
3.278 The medium I is vacuum and contains a circular current carrying coil with cunent L The medium П is a magnetic with permeability ц. The boundary is the plane z = 0 and the coil is in the plane z = /.To find the magnetic induction, we note that the effect of the magnetic medium can be written as due to an image coil in II as far as the medium I is concerned. On the other hand, the induction in II can be written as due to the coil in I, carrying a different current. It is sufficient to consider the far away fields and ensure that the boundary conditions are satisfied there. Now for actual coil in medium Z,
where	pm = Z(it a2), a = radius of the coil.
Similarly due to the image coil,
В =	(2 COS2 0' - sin2 0'), Bx =	(3 sin 0' cos 0'), p'm = Г (л a )
z 4л	* 4л	nt m \	/
As far as the medium II is concerned, we write similarly
В =	(2 cos2 0' - sin2 0'), Bx =	(- 3 sin 0' cos &\p"m = Iй (я a2)
z 4л;	4л
378
The boundary conditions are, pm + p'm « p"m (from B^n «
-Pm+P'm= - T7P"m (from Hu = Я*) И
Thus,	I" - I, Г -	4-/
Ц + 1	Ц + 1
In the limit, when the coil is on the boundary, the magnetic field enverywhere can be obtained by taking the cunent to be —- /. Thus, В = ц+1	ц + 1 u
3.279
3.280
3.281
—-J/3 Ho
We use the fact that within an isolated uniformly magnetized ball, -*	2ц0 J
H - - J/3, В - —-—, where J is the magnetization vector. Then in a uniform magnetic field with induction Bo, we have by superposition, —>	—*	J	—*
or, К, + 2|10Я,= зв’о^ also, tf	я n*
ж —;-----тгг and B- » -----—
ц0(ц + 2) m Ц + 2
Thus,
The coercive force Hc is just the magnetic field within the cylinder. This is by circulation
NI
theorem, Hc = — = 6 kA/m
(from ф Hdr= I, total current, considering a rectangular contour.)
We use, ф H-dl = 0
Neglecting the fringing of the lines of force, we write this as
H{nd-b) + — b = 0
Ho
or,	——t - 101 A/m
The sense of H is opposite to В
r -» -»	Bb	NIpn-Bb
3.282	Here,® H-dl^NI or, H(2kR) + — - NI, so, Я- —-----
J	Ho	2 л R p.Q
„	В 2nRB „nn
Hence,	ц = —- = —	- - 3700
ц0Н n0NI-Bb
p
3.283	One has to draw the graph of ц = —— versus H from the given graph. The ц - H graph Ho7*
starts out horizontally, and then rises steeply at about H = 0-04 к A/m before falling agian.
It is easy to check that цтах « 10,000.
379
3.284	From the theorem on circulation of vector H .
Bb	Hn^l Ho^d
Hitdi — -Nl or, B-	(1-51-0-987)77,
Ho	b b
where В is in Tesla and H in кА/m. Besides, В and H are interrelated as in the Fig. 3.76 of the text. Thus we have to solve for В, H graphically by simultaneously drawing the two curves (the hysterisis curve and the straight line, given above) and find the point of intersection. It is at
Then,
3.285	From the formula,
F= (j£-V pj (J* V)BdV,
H~ 0-26 кА/m, В - 1 H- -^77- 4000.
Thus
F- I (B-V)BdV
or since В is predominantly along the x-axis, °- Sx 2uH„xJ0 « 2цИ„ 2И<|
F. -
3.286	The force in question is,
(£• =
HHo
dB dx
since В is essentially in the x-direction.
_	vVdB2 XBo2V d . -2ax\	.
So,	Г---T~ = —---v(e )- -4axe
2 p0 dx 2 Ho dx
-2дх2
2^o
This is maximum when its derivative vanishes
i.e.	16 a2 x2 - 4 a - 0, or, x„ = J—
7	7	< / Л
The maximum force is,
F 4*	e'»2*^
m“ VS7 2 p0
Ho ' e
So, X- Iho^VFJ / VB°2 ‘ 3’6 X 10’4


3.287
xBVdB _ dB2 ц p0 dx 2 p0 dx
This force is attractive and an equal force must be applied for balance. The work done by applied forces is, L
A= f -Fxdx=
J x 2 Ho x °	2 Ho
X- 0
380
3.6
ELECTROMAGNETIC INDUCTION. MAXWELL’S EQUATIONS
3.288
Obviously, from Lenz’s law, the induced cunent and hence the induced e.m.f. in the loop is anticlockwise.
-(vxB)-dl
= vBl

_—>
and directed in the same of (v x B) (Fig.)
__A_
A + -_ 1 2 Я1+Я2
So,
Bvl
R1R2
Rr+Rz
3.290
As and R2 are in parallel connections.
(a) As the metal disc rotates, any free electron also rotates with it with same angular velocity co, and that’s why an electron must have an acceleration co2r directed towards the disc’s centre, where r is separation of the electron from the centre of the disc. We know from Newton’s second law that if a particle has some acceleration then there must be a net effecetive force on it in the direction of acceleration. We also know that a charged particle can be influenced by two fields electric and magnetic. In our problem magnetic field is absent hence we reach at the conslusion that there is an electric field near any electron and is directed opposite to the acceleration of the electron.
If E be the electric field strength at a distance r from the centre of the disc, we have from Newton’s second law.
F = mw„ n	n
eE = m r co2, or, E
mod2 r e
and the potential difference,
Ween ^rim
mw2r ,	, -
-----ar, as E f j d r e
о
0
381
2 2 . wicu a . n Thus	Фсе„-<Р™= Лф= “7“7= 3‘0nV
—>
(b) When field В is present, by definition, of motional e.m.f. : 2
Ф1-Ф2- J' -(v^B')-dr* 1
Hence the sought potential difference, a	' a
f-vBdr~ J* - w г В dr, (as v = <or) о	0
1	7
Thus	<P™-<Pcen= <P=	= 20 mV
eB
(In general co < — so we can neglect the effect discussed in (1) here). nt
3.291 By definition, £*» -(Zx?)
C	C	d
Sq,	J*E-dr^j* - ( 7хВ ) • dr*= J* -vBdr
а	а	о
But, v = cor, where r is the perpendicular distance of the point from A. c	d
/->	_> r	1 о
Edr = J -coBrdr= - —co В d2 = - 10 mV A	0
This result can be generalized to a wire AC of arbitary planar shape. We have c	c	c
J* E • dr =	( v x B) • dr •	((co x r) x B) • dr
A	A	A
(В • r (o~B-(o r)- dr
-^Btad2, 2
d being AC and r being measured from A.
3.292 Flux at any moment of time, |ф,|= |F-t/sj=
where ф is the sector angle, enclosed by the field. Now, magnitude of induced e.m.f. is given by,
382
dQ, BR2dq> BR2
’	°	*	’	" 2

2 dt
dt
where co is the angular velocity of the disc. But as it starts rotating from rest at t« 0 with an angular acceleration 0 its angular velocity co (f)« 0Г. So,
According to Lenz law the first half cycle current in the loop is in anticlockwise sense, and in subsequent half cycle it is in clockwise sense.
В
Thus in general, - (- 1)Л —-— 0 r, where n in number of half revolutions.
The plot (r), where tn = V2 л и/0 is shown in the answer sheet
3.293
3.294
Field, due to the current carrying wire in the region, right to it, is directed into the plane of the paper and its magnitude is given by,
Ho i
В = -— where r is the perpendicular distance from the wire. 2л r
As В is same along the length of the rod thus motional e.m.f.
2
lin
vBl
1
and it is directed in the sense of (vxВ )
So, current (induced) in the loop,
1 ^Ivi R 2 nRr

Field, due to the current carrying wire, at a perpendicular distance x from it is given by,
s(x>-
2л x
Motional e.m.f is given by
f -(v*B)-dF
There will be no induced e.m.f. in the segments (2) and (4) as, П t d I and magnitude of e.m.f. induced in 1 and 3, will be
5i= v ---la and « v
12лх Ъ2
Ho i 2л (а + х)
respectively, and their sense will be in the direction of ( v x В ).
So, e.m.f., induced in the network =	- %2 [as > ^2 J
д УЦр» r 1. _ 1 1 _ V f? Ho i
2л x a+ x 2 л x (a + x)
383
3.295 As the rod rotates, an emf.
y-^a2e-B“ ^a2B(0 dt 2	2
^(r)-|a2Bco
is induced in it. The net current in the conductor is then
R
A magnetic force will then act on the conductor of magnitude BI per unit length. Its direction will be normal to В and the rod and its torque will be
о
/ 1 ?
?(г)-|а2Бсо
dxBx
Obviously both magnetic and mechanical torque acting on the C.M. of the rod must be equal but opposite in sense. Then for equilibrium at constant co
------J---------Y~'" 2mSasm(Ot
or, £ (r) = a2 В co + sin co t = —Цг (a3 B2 co + 2 mg R sin co t) 2	aB	2 aB
(The answer given in the book is incorrect dimensionally.)
3.296
From Lenz’s law, the current through the connector is directed form A to B. Here = vBl between A and В
where v is the velocity of the rod at any moment.
For the rod, from Fx = mwx
or, mg sin a - i IВ « mw
For steady state, acceleration of the rod must be equal to zero.
Hence, mg sin a = i IВ	(1)
_ z.s . /-x тир sin a Я From (1) and (2) v = -£—— В I
3.297 From Lenz’s 1 aw, the current through the copper bar is directed from 1 to 2 or in other words, the induced crrrent in the circuit is in clockwise
sense.
Potential difference across the capacitor plates, lin or> 9=
384
and,
Hence, the induced current in the loop, dt dt
But the variation of magnetic flux through the loop is caused by the movement of the bar.
So, the induced e.m.f. = В Iv
—^= Bl^ = Blw dt dt
Hence,
= CBlw dt
Now, the forces acting on the bars are the weight and the Ampere’s force, where Famp= i IB (CBlw) B- Cl2B2w.
From Newton’s second law, for the rod, Fx = mwx or,
mgsina-C l2B2 w = mw mg sin a______gsin a
W~ Cl2B2 + m~ л l2B2C tn
Hence
1298 Flux of B, at an arbitrary moment of time t : .	2
у	y. ~ y. TC Cl
Фг = В ' S = В —— cos co r,
d$
From Faraday’s law, induced e.m.f.,
L «2	1
, В л — cos cot d\	2	I	n 2
x	7 В ла co .
—----sm co t.
dt
. . .	.	.	. ^>in В л а
and induced current, i- = — = ——— co sm co t.
’ m R 2R ,
Now thermal power, generated in the circuit, at the moment t = t : t 2 \2
s-	В ла co) 1 . 2	.
P(t) = x I;- = --------~~ Sin CO t
v '	in I о I d
and mean thermal power generated, 2 T
— I sin2 co t dt К J 0 T
В л a2 cd
2
1
Лгсо а2 В\
о
Note : The ejaculation of which can also be checked by using motional emf is correct even though the conductor is not a closed semicircle , for the flux linked to the rectangular part containing the resistance R is not changing. The answer given in the book is off by a factor 1/4.
385
3.299 The flux through the coil changes sign. Initially it is BS per turn.
Finally it is - BS per turn. Now if flux is Ф at an intermediate state then the current at that moment will be
. dt R
So charge that flows during a sudden turning of the coil is ;N
idt~ -~[Ф-(-Ф)] « 2NBS/R R
Непсе,
B = —	• 0’5 T on putting the values.
а
o'
3.300 According to Ohm’s law and Faraday’s law of induction, the current iQ appearing in the frame, during its rotation, is determined by the formula, d Ф L d iQ ‘° ° ~ dt " " dt
Hence, the required amount of electricity (charge) is, q~ f iodt- f И +	-|(ДФ+£Д/0)
Since the frame has been stopped after rotation, the cunent in it vanishes, and hence A /0= 0. It remains for us to find the increment of the flux А Ф through the frame (А Ф » Ф2 - Фх).
Let us choose the normal n to the plane of the frame, for instance, so that in the final position, n*is directed behind the plane of the figure (along В ).
• Then it can be easily seen that in the final position, Ф2 > 0, while in the initial position, Фх <0 (the normal is opposite to В ), and АФ turns out to be simply equal to the fulx through the surface bounded by the final and initial positions of the frame :
b + a

ДФ
В a dr, b-a where В is a function of r, whose form can be easily found with the help of the theorem of circulation. Finally omitting the minus sign, we obtain, АФ	Ho a *'. b + a
q‘ R ‘ 2лЛ П b-a
3301 As В, due to the straight current carrying wire, varies along the rod (connector) and enters linerarly so, to make the calculations simple, В is made constant by taking its average value in the range [a, 6].
386
(a) The flux of В changes through the loop due to the movement of the connector. According to Lenz’s law, the current in the loop will be anticlockwise. The magnitude of motional e.rn.f.,
v<B>(b-a)
Ио <o , b „ Xdx  , b 2 л (b - a) a dt 2 л 0 a
So, induced current lin Ио lov) b l“=Y‘ 2^Tlna
(b) The force required to maintain the constant velocity of the connector must be the magnitude equal to that of Ampere’s acting on the connector, but in opposite direction.
„ г» • t D	*o 1 M	M
So,	-— — vln— (b-fl) -—T,-----------?ln —
’	w	^2 л R aj ' \2 л (b-a) a)
2
-	1<o In ~ | » and will be directed as shown in the (Fig.)
R 12 л a I
3302 (a) The flux through the loop changes due to the movement of the rod AS. Recording to Lenz’s law current should be anticlockwise in sense as we have assumed В is directed into the plane of the loop. The motion e.rn.f ^(r) « В Iv
and induced current =
vBl R
From Newton’s law in projection form Fx = mwx
„	vdv
m—— omp	fa
But
F*® L.IB» Q/Пр Ut
vB2l2 R
So,
vB2/2 R
mv —
387
or,
x	О
r mR Г
1 ах - - —z—г I dv or,
< в i2J
°	vo
mRv0 в212
(b) From equation of energy conservation; Ef - E{ + Heat liberated « Ace// + Aa
0-2mV°2
+ Heat liberated
0 + 0
1	2
So, heat liberated = — m v0
3.303 With the help of the calculation, done in the previous problem, Ampere’s force on the connector, vB2l2
Fnmn = —”— directed towards left. amp • д
Now from Newton’s second law,
F - F = amp &
F =
So,
or,
vB2!2	dv
—-— + m — R	dt
m
о
Г dv
J „ vB2l2
0F—r-
or,
t R , — = --3-77 In m	B2l2
F-^-
Thus
Л -tB2l2\ RF Rm ) B2l2
3304 According to Lenz, the sense of induced e.m.f. is such that it opposes the cause of change of flux. In our problem, magnetic field is directed away from the reader and is diminishing.
(<x)	(b)
So, in figure (a), in the round conductor, it is clockwise and there is no cunent in the connector
In figure (b) in the outside conductor, clockwise.
388
In figure (c) in both the conductor, clockwise; and there is no current in the connector to obey the charge conservation. In figure (d) in the left side of the figure, clockwise.
3.305	The loops are connected in such a way that if the current is clockwise in one, it is anticlockwise in the other. Hence the e.m.f. in loop b opposes the e.m.f. in loop a. e.m.f. in loop a = ~ (a1 2 B) = a2 ~ (BQ sin cor)
Similarly, e.m.f. in loop b == b2 BQ co cos cor.
Hence, net e.m.f. in the circuit  (a2 - b2) BQ co cos cor, as both the e.m.f’s are in opposite
sense, and resistance of the circuit = 4 (a + b) p
Therefore, the amplitude of the current
(«2-Ь2)Б0со = Чт—тг— 0-5 A. 4 (a + b) p
3.306	The flat shape is made up of concentric loops, having different radii, varying from 0 to a. Let us consider an elementary loop of radius r, then e.m.f. induced due to this loop
-J(ZF) 2n = -----------= я r Д (O COS
dt	0
and the total induced e.m.f.,
^>ind ~ J* (л r 2B0 co cos cor) dN,	(1)
0
where л r2 co cos cor is the contribution of one turn of radius r and dN is the number of turns in the interval [r, r + dr].
So,	d#-	(2)
a 2 hi л Bn co cos co rN a
- (л r BQ co cos cor) — Jr « ------------------------
0
1	2
Maximum value of e.m.f. amplitude §max= — nBowN a
3.307
The flux through the loop changes due to the variation in В with time and also due to the movement of the connector.
dCB-S*) dt
So,
d(BS) dt
as S and В are colliniear
1	2
But, B, after r sec. of beginning of motion - Bt, and 5 becomes « I — w t , as connector
starts moving from rest with a constant acceleration ж
So,
389
3.308 We use В = ц0 n I
Then, from the law of electromagnetic induction f E-dT--d-± J	dt
So, for r < a
Еф2лг= -лг ponZ or> ” 2 (where Z - dl/dt)
For r>a
Ey2nr= -ъа2\^п1 or, Еф= -ц0п/а2/2г
The meaning of minus sign can be deduced from Lenz’s law.
• d2
3.309	The e.m.f. induced in the turn is —
.	. л d
The resistance is —- p.
\kQnISd
S(y, the current is ——— = 2 m A, where p is the resistivity of copper.
3.310	The changing magnetic field will induce an e.m.f. in the ring, which is obviously equal, in the two parts by symmetry (the e.m.f. induced by electromagnetic induction does not depend on resistance). The current, that will flow due to this, will be different in the two parts. This will cause an acceleration of charge, leading to the setting up of an electric field E which has opposite sign in the two parts. Thus,
^-лаЕ= rl and, +	= nrZ,
where is the total induced e.m.f. From this,
S=(n + I)rf,
and	£= —(r]-l)rf=	2L~H
2 л а	2лдт] + 1
2
But by Faraday’s law,^ « л a b
so,	E = ab B-Z-l
2 T] + 1
3.311 Go to the rotating frame with an instantaneous angular velocity aT(r). In this frame, a Coriolis force, 2m v x co (r)
acts which must be balanced by the magnetic force, e v x В (t\
Thus,	.
2 m
(It is assumed that co is small and varies slowly, so co and co can be neglected.)
390
3.312 The solenoid has an inductance,
L = ц0и2 лЬ2/,
where n = number of turns of the solenoid per unit length. When the solenoid is connected to the source an e.m.f. is set up, which, because of the inductance and resistance, rises slowly, according to the equation,
AZ + jL7= V
This has the well known solution,
1= ^(l-e-,K/£). I\
Corresponding to this current, an e.m.f. is induced in the ring. Its magnetic field
dF .	22	2
В - |i0 n I in the solenoid, produces a force per unit length, — = В i= ц0 n тс а II/ r
2	2 vz 2 / о \
До Л fl V / n \ -tR/L Z« _ -tR/L\ r	U	Л
acting on each segment of the ring. This force is zero initially and zero for large t. Its maximum value is for some finite t. The maximum value of
So
e~,R/L(l-e-,R/L)=	is |.
dFmax_ £*a2V2 n2 _ Ho«2^2
dl	r 4RL 4rRlb2
3.313
3.314
The amount of heat generated in the loop during a small time interval dt, dQ- l2/Rdt, but, § -	2 at-ат,
So,
dQ-
К
and hence, the amount of heat, generated in the loop during the time interval 0 to t.
/(2 at-ax)2 .	1 a2t3
' R эПГ 0
Take an elementary ring of radius r and width dr. The e.m.f. induced in this elementary ring is n r2 p. Now the conductance of this ring is. hdr	hr dr Q
If? I р2лг	2p
Integrating we get the total current, b
hr dr Q hfi (b2 - fl2)
ТГ'" 4p
391
2	2	2
3.315 Given L = ЦцП V= v^n l^R , where J? is the radius of the solenoid.
Thus,
VL 1
Ho 31 &
So, length of the wire required is,
I = n lQ 2 itR =
4 TtL L ------= O-lOkm.
Ho
3316 From the previous problem, we know that,
/' = length of the wire needed-	> where I~ length of solenoid here.
Po I'
Now, R = ——, (where S = area of crossection of the wire. Also m = p 5 I) О
Thus,
RS R m Po P Po ?
T =
where p0 = resistivity of copper and p = its density.
Equating, or,
Rm LI
PPo Ho/4 31
~ 4 я pp01
3.317 The current at time t is given by,
IX
The steady state value is, /0 «
V R
and
70
R ,	1	L.	1 i
3318 The time constant т is given by
L .1— x~ R~ V po5 where, p0 « resistivity, lQ - length of the winding wire, 5 « cross section of the wire. But	m = I Po S
So eliminating 5,t = -----— = --------y
Po'o PPoV m/p/0
392
3.319
3.320
From problem 3.315 /0 = у —— Ио
(note the interchange of I and /0 because of difference in notation here.) ml	л m
----------= цп4тг------= 0-7 ms,
OD 12bz PPo*
PPo .. b 1 Ио
Thus,
Between the cables, where a < r < b, the magnetic field H satisfies
Hv2xr= I or,
2 л r
So
2л г
The associated flux per unit length is,0 =
r= a
HHoJ J нМ, b
—---X 1 X dr = —-In —
2лг	2л a
Ф	ИИо	b
Hence, the inductance per unit length Lr = — = -—In r), Where r] = — 1	2 л	a
We get L, = 0-26 1	m
Nl	Nl
Within the solenoid Я-2 лг= Nl or H = *	* 2 л г	*	2 л r
and the Аих,Ф = N&p = N
b
393
3.322
Но
For a single current carrying wire,# « ----(r > a). For the double line cable, with current,
ф 2 'л r
flowing in opposite directions, in the two conductors,
Ho/
® -^7, between the cables, by superposition. The associated flux is, d-a
drxl M, J Ho. T . ,	.
-----------® -----In — = — In ri x L per unit length л г л а л
Hence,	L, = —Inn
1 л
is the inductance per unit length.
3.323 In a superconductor there is no resistance, Hence, d7= d® dt + dt
So integrating,	/=	—7—
JL	Lt
because	АФ = Фу - Ф;, Фу = л а2 В, Ф; = О
Also, the work done is, A = I ^Idt =
3.324
yr LI2 2
IrcVl2
2 L
N 2 S
In a solenoid, the inductance L = HHo n V = н Ho —j—,
where 5 « area of cross section of the solenoid, I« its length, V « SI, N « nl = total number of turns.
When the length of the solenoid is increased, for example, by pulling it, its inductance will decrease. If the current remains unchanged, the flux, linked to the solenoid, will also decrease. An induced e.m.f. will then come into play, which by Lenz’s law will try to oppose the decrease of flux, for example, by increasing the current. In the superconducting state the flux will not change and so,
constant
3.325
Hence,
or, /=/02-=/0(l+n)
L l0	Z0
The flux linked to the ring can not change on transition to the superconduction stale, for reasons, similar to that given above. Thus a current I must be induced in the ring, where, _ Ф л a2B лаВ
L A 8a А 8л p°a lny-2 H0 lln —-21
394
3326
We write the equation of the circuit as,
T) dt ъ
for Г £ 0. The current at t  0 just after inductance is changed, is
5
i - Л d ’ so that the flux through the inductance is unchanged. R
We look for a solution of the above equation in the form
i = A + Be t/c
1
R
i- ^1 + (r) - 1) e~nRt/L )
Substituting C «	,B- t) - 1,A
Thus,
3.327
Clearly, L R(I-i) = ?-Rl
So, 2L^- = t-Ri dt ’
This equation has the solution (as in 3.312)
i= 1(1-е-'л/2£) Z\
3.328
The equations are, di* din
Then, ~ (L1 ij — i^) = 0
Lt
J.329
Л
h Lz
or, Lr -L2i2 = constant
But initially at t« 0,	“ 0
so constant must be zero and at all times, h ~ ^>2 h
W2
ъ
In the final steady atate, current must obviously be ir +	• Thus in steady state,
• _	^2	. . _ __!b_
h ACL.+jL,) ana 12 R (Li+LJ
Неге, В = -— at a distance r from the wire. The flux through the frame is obtained as, 2 лт
a +1
ф12 = J I
Mo^ bdr = ^-1 In 2л
Thus, L12 =
ф12 h/*	/ a'
1 + 7
a
395
ь
3.330
тт 1 D	,	/ Г hdr fcr
Here also, В « -—and Ф= цпцт— I -----AT-
’	2 яг	r°r2nJ r
Thus,
^12 “
b
—------In —
2л a
3.331
The direct calculation of the flux Ф2 is a rather complicated problem, since the configuration of the field itself is complicated. However, the application of the reciprocity theorem simplifies the solution of the problem. Indeed, let the same current i flow through loop 2. Then the magnetic flux created by this cunent through loop 1 can be easily found.
p0*
Magnetic induction at the centre of the loop, : В =	—
2b
2 Ho4
So, flux throug loop 1, : Ф12 = тс a
and from reciprocity theorem, Po я a'i
Ф12 = Ф21 > Ф21 = —2b—
So, L12 =	| Mq л a2/b
3332
Let pm be the magnetic moment of the magnet M. Then the magnetic field due to this magnet is,
Но
4я r5 r3
The flux associated with this, when the magnet is along the axis at a distance x from the centre, is
a
r5 r3
dS- Фх - Ф2.
where,Ф2 =
4TCPmJ
2 я pJ p (x2 + p2)3/2 =
a
3 popmx2 «	r U •* m
and Ф, = --------
1	4л
I 2 я ptZ p J (? + p2)5/2 0
1 1
2	P3'(x2 + a2)3/2
- PoPM
г» /к	r m
^oP„ p l \ 2 p Vx2 + a2 ,
396
d ф	d Ф
When the flux changes, an e.m.f. - N —— is induced and a current - — —r~ flows. The dt	R dt
total charge q, flowing, as the magnet is removed to infinity from x = 0 is, PoPm
2a
N
q- ~^ф(х = °) 1\
or,
N
R
p =
^Po
3.333
3.334
If a current / flows in one of the coils, the magnetic field at the centre of the other coil is,
Poa I Poa - •
В = ---з----7^77 - ----T-, as I» a.
2(l2 + a2)3/2	213
The flux associated with the second coil is then approximately ц0ла4//2/3
_ Po я a4
12 “ 2/3
Hence,
dIY
When the current in one of the loop is = ar, an e.m.f. L12~^~ - B12a, is induced in
the other loop. Then if the current in the other loop is I2 we must have,
dl2
^2	+	= ^12 a
This familiar equation has the solution,
-^12 tX /	£	\
I2 = ———11 -e 2 I which is the required current
3.335 Initially, after a steady current is set up, the current is flowing as shown.
In steady condition z20 = •*, z10 = -g- • л	Kq
When the switch is disconnected, the current through Rq changes from i10 to the right, to z20 to the left. (The current in the inductance cannot change suddenly.). We then have the equation, di.
L ~ +(R+R0) i2 = 0.
This equation has the solution i^e
The heat dissipated in the coil is,
Q = f i2Rdt = i^R J* e~2s(R + R«yL dt о	о
D -2	1	L^2	. T
“ л'20 x 2(R + R0)“ 2R(R+R0)“
397
3.336 To find the magnetic field eneigy we recall that the flux varies linearly with current Thus, when the flux is Ф for cunent i, we can write ф = A L The total energy inclosed in the field, when the cunent is I, is
W- J t-idt-JN ^-idt
I
/С	lol
Nd®i-J NAidi- ±NAI- ~N<M о
The characteristic factor ~ appears in this way.
3.337 We apply circulation theorem,
H2 n b » NI, or, H = NI/2 nb.
Thus the total energy,
W= ~ВН-2яЬла2~ п2а2ЪВН.
2
Given N, I, b we know H, and can find out В from the В - H curve. Then W can be calculated.
3.338 FromHdr*= NI,
H-nd + —b~ NI,(d»b) Ho
Also,
NI
B =	Thus, H =
u	nd + po
Since В is continuous across the gap, В is given by,
B =
(a)
NI p p0 —  * — , both in the magnetic and the gap.
В2 с к
-—x 5 x b
2 Ho jxb
B2	nd ‘
-----xS x nd 2цц0
W ____ F. spiv magnetic
/_>	NT	S N2 T
B-dS = Niwq -S = p0------7
u nd+ub u , nd r	b +—
H
So,
L &
HqSN2 . nd * b + —
H
Energy wise; total energy
B2(nd — I----h b IЛ = ~ - ————
2|10( Ц )	2 b + —
H
Il/’
398
The L, found in the one way, agrees with that, found in the other way. Note that, in calculating the flux, we do not consider the field in the gap, since it is not linked to the winding. But the total energy includes that of the gap.
3.339 When the cylinder with a linear charge density к rotates with a circular frequency co, a surface current density (charge I length x time) of Xco .
i = — is set up.
2л F
The direction of the surface current is normal to the plane of paper at Q and the contribution of this current to the magnetic field at P is
Ho i (ex r)	_
dB =  -----—;—LdS where e is the
4 л /
direction of the current. In magnitude, |exr^ = r, since e*is normal to F’and the —>
direction of dB is as shown.
—>
It’s component, d Бц cancels out by cylindrical symmetry. The component that survives is,
|Б>. Ho f idS Q Ho2 Г
I5x|=	-^-cos0= —J dQ = Иог,
where we have used	= d Q and Г d Q = 4 л, the total solid angle around any
r	J
point.
The magnetic field vanishes outside the cylinder by similar argument.
The total energy per unit length of the cylinder is,
z ц0
2
1	2 Но 2л2 2
x л a - —a A cd
I	8л
1	2
3.340	= — e0 E , for the electric field,
1	2
wB = ----В for the magnetic field.
2
Thus,	-i- В2-\г0Е2,
2ц0	2 0
В	R
when	E « -7== = 3 x 108 V/m
ve0 Цо
3.341 The electric field at P is, F -	ql
p~ 4 л e0(a2 + /2)3/2
399
3342
To get the magnetic field, note that the rotating ring constitutes a current i« q co/2 л, and the corresponding magnetic field at P is,
The total eneigy of the magnetic field is,
(BH)dV- | f b"-(— dV
2 J	2 J j
- 7Г- f
2 Ho J	2J
The second term can be interpreted as the energy of magnetization, and has the density
3.343 (a) In series, the current I flows through both coils, and the total e.m.f. induced, when the current changes is,
-2L^-~ ~L'^ dt dt
or,	L' = 2L
(b) In parallel, the current flowing through either coil is, and the e.m.f. induced is
/1 4L\
L \2 dt /
Equating this to - L' , we find L' =	.
at	2
3344 We use Lx - h0 nx2 V,L2 - Ио «г2 v )
So,	^12* Нои1и2^“ ^B^L2
3345 The interaction energy is
f [B^B^dV-
2 Ho I I 2 |Aq
—> 2
в, I dV-
2 dV
= — f -B,dV
Ho J 1 2 —>
Here, if BT is the magnetic field produced by the first of the current carrying loops, and B2, that of the second one, then the magnetic field due to both the loops will be + B2.
400
3.346 We can think of the smaller coil as constituting a magnet of dipole moment,
Pm“
Its direction is normal to the loop and makes an angle G with the direction of the magnetic field, due to the bigger loop. This magnetic field is,
Б2- 2b
The interaction eneigy has the magnitude,
iwq-
2b
л a2 cos 0
Its sign depends on the sense of the currents.
= "J
3.347 (a) There is a radial outward conduction current Let Q be the instantaneous charge on the inner sphere, then,
j x 4 л r = - — or, j  J	dt J
t* dD	d I Q a
On the other hand = — = — ----------7 r
*	dt	dt |4ЛГ2
—*	n л
(b) At the given moment, E = -------3----7 r
4 л e0 e r
—*
—* E	q л
and by Ohm’s lawj = — « -----3------« r
P 4 л e0 e p r
Then,
Id* ~л—2—J 4 л г0 e pH
, Ж jT* q Г dS cose q
and	(D jd • dS - - -—3----- I -----5---------.
J “	4jt£0£pJ /	£0£p
The surface integral must be -ve because jd, being opposite of j, is inward.
—•>	—>
3.348 Here also we see that neglecting edge effects, jd - - j. Thus Maxwell’s equations reduce to,div В = 0, Curl 27 = О, В « [iH
A general solution of this equation is В » constant  Bo • BQ can be thought of as an
—>
extraneous magnetic field. If it is zero, В = 0.
3.349 Given I = I sin oor. We see that m
.	. t	dD
or, D = —~ cos oof, so, = ----------- is the amplitude of the electric field and is
co S	m eocoS
7V/cm
401
3350 The electric field between the plates can be written as, V	V
E « Re —£ instead of —7^ cos cor. a	a
This gives rise to a conduction current,
jc= aE= Re^VmeiM
and a displacement current, dD o	ion
Ree0eico — e
The total current is, jT« -—Va2 + (e0 e co)2 cos (co r + a)
where, tan a = on taking the real part of the resultant.
The corresponding magnetic field is obtained by using circulation theorem, Я’2лг = лг2;г
rVn r~z-----------5"
or, H = Ят cos (cor + a), where, Hm = Va + (eoe co)
3351 Inside the solenoid, there is a magnetic field, В « ц0 n Im sin cor.
Since this varies in time there is an associated electric field. This is obtained by using,
(j)	Edr*- J B’dS*
c	s
For
For
* о	В r
r< R, 2тсгЕ- -В'лг, or, E» - — ’	’	’	2
r > R,E-
BR2 2r
The associated displacement current density is, - e0 В r/2 -t0B R2/2 r
dE }d~ «о dt
The answer, given in the book, is dimensionally incorrect without the factor Eq.
3352 In the non-relativistic limit
4 Л Eq Г
(3)
On a straight line coinciding with the chaige path,
—_ iA JL }“~ e° dt = 4л
-V 3rr r4
f . dr
using, T =
V.
402
D . . ...	•	. r -»	2qv
But in this case, r - - v and v - » v, so, ij - — r	4 nr
(b) In this case,r« 0, as, rl. v^Thus,
4л?
3353
We have, EB  --------?—тт,
p 4ле0(«2+х2)3/2
. dD dE ______________qv________, 2 о 2\
1^еП	idж ж £° ж A ( 2	2\5/2 (a ~	)
dr dt 4л(а +x )
This is maximum, when x « xm « 0, and minimum at some other value. The maximum displacement current density is
4d)am 4 ла3
djd
To check this we calculate —— ; dx
This vanishes for x »
/3
0 and for x  у — a. The latter is easily shown to be a smaller
local minimum (negative maximum).
3354 We use Maxwell’s equations in the form,
f В-dF*- еоИо£ f E dF,
where, 2л (1 - cos 0) is the solid angle, formed by the disc like surface, at the charge.
Thus,	(|> В -dr^ 2 л a В - ~ p0 q • sin 0 • 0
On the other hand,* * a cot 0 differentiating and using « - v, v « a cosec2 0 0
Thus,
p0 v r sin 0
4я?
403
Ho Q (v x H
This can be written as, В « -------z—
4 nr3
and Я =	(The sense has to be checked independently.)
4 Л
3355
(a) If В = В (r), then,
Curl £=-^*0. dt
So, E cannot vanish.
(b)	Here also, curl E * 0, so E cannot be uniform.
—>
(c)	Suppose for instance, E « a f (t)
—>
—>	dB	—♦
where a* is spatially and temporally fixed vector. Then - — = curl E » 0. Generally
—>
speaking this contradicts the other equation curl H = — * 0 for in this case the left
hand side is time independent but RHS. depends on time. The only exception is when f (r) is linear function. Then a uniform field E can be time dependent.
—>
dD t*
3356 From the equation Curl H - —  j
dt
We get on taking divergence of both sides d —-- —- div D = div j dt	J
But div D = p and hence div j +	« 0
dt
3357
_ z* z* dB
From V x E = - — dt
we get on taking divergence
0= --T-divi? t dt
This is compatible with div В » 0
3358 A rotating magnetic field can be represented by, Bx = Bo cos co t; By = Bo sin cor and Bz • Bw
Then curl, E - -	.
’	dt
—>
So,	-(Curl£)x® - соЛ0 sin cor = -coBy
- (Curl E )y = co jB0 cos cor « cofix and - (Curl E )z * 0
Hence, Curl E  - co x В ,
where,	co « e3 co .
404
3359 Consider a particle with charge e, moving with velocity v, in frame K. It experiences a force F = evxB*
In the frame K9, moving with velocity v^ relative to K, the particle is at rest. This means that there must be an electric field E in JT, so that the particle experinces aforce,
F' - e E' « F » e vx В
Thus,	Ef - vxВ
3360 Within the plate, there will appear a (v x В) force, which will cause charges inside the plate to drift, until a countervailing electric field is set up. This electric field is related to B, by Е» eB, since v&B are mutually perpendicular, and E is perpendicular to both. The charge density ± a, on the force of the plate, producing this electric field, is given by
E » — or a « e0 v В « 0-40 pC/m2 eo
3361 Choose (0 11 В along the z-axis, and choose r, as the cylindrical polar radius vector of a reference point (perpendicular distance from the axis). This point has the velocity,
V = (0 X r, > and experiences a (v x В ) force, which must be counterbalanced by an electric field, £« -(wxr^xB - -(oT-B)rT
There must appear a space charge density,
p» eodiv Е» -2е0(йГ-В » -8pC/m3
3362
Since the cylinder, as a whole is electrically neutral, the surface of the cylinder must acquire a positive charge of surface density,
2 Ел («• В) ла2 » —>	~
a = +---------------- e0 a co • В « + -2 pC/m2
2ла	0	r
In the reference frame K, moving with the particle,
E' « E + Vo x В - — 4 л e0 r
Bf л B-v^xE /с2 = 0.
Here, « velocity of Kf, relative to the К frame, in which the particle has velocity vT
Clearly, Vq = v^From the second equation, vx E	q vx r* Ho q (vx r}
В “ —eoHox л-------------—
c2 u u 4 я e0 г3 4л r3
405
3363 Suppose, there is only electric field E, in K. Then in K', considering nonrelativistic velocity
F, B*= c	» >
So,	£'B'=0
In the relativistic case, £'ll= £ll _» B'll ~BII~ к-,£1	.	- ioc E/c2
V 1 - v2/c2	4\-v2/c2
Now,
E’-B'= E'ii • B'ii + Ef L -B'± « 0, since
-Ex-(v*xE)/(l-v2/c2) = -Е^(у*Е^ )/ |l--^j = 0
3364 In 7С,1Г- byl~Xl, b- constant X +У	л
In	K, E = ЛВ= bv УI ~X~ = bv
XT + yr	r
л л The electric field is radial (r = xi + yj ).
3365 InAj£= a~, Г= (xi+yj ) r	ш
vxE arxv* m	к-л - - — -
The magnetic lines are circular.
3366 In the non relativistic limit, we neglect v2/c2 and write, = В . _»1 в и=	-»
 El + vxВ	-vxE/c2
These two equations can be combined to give, F- F+v*xF, T-v^E/c2 —♦	—>
3367 Choose E in the direction of the z-axis, E » (0,0, E). The frame K( is moving with velocity v = (v sin a, 0, v cos a), in the x - z plane. Then in the frame Kf,
E'\\~EiB'r °
p - Ej- Tv -vxE/c2 x’ х" ГГм
The vector along v*is ё^= (sin a, 0, cos a) and the perpendicular vector in the x - z plane is,
/= (- cos a, 0, sin a),
406
(a)	Thus using E = E cos a e + E sin a f t
Esina
E и - E cos a and E n - z   11 - —,
11	11 VTT?
о	\/1 - ₽2 cos2 a	j , tan a
So	E » E V--c—Й  and tan a » -====
l-₽2	Vl-v2/c2
__> 2
(b)	B',| = 0, ? = vx^/-c--
B,= pEsina cVl-p2
3.368 Choose В in the z direction, and the velocity (v sin a, 0, v cos a) in the x - z plane,
then in the K’ frame,
£'ll  д|| -»
-Г	BL
B\- , VI - vVc2
We find similarly, E' - C P — sS-g-vr?
„ Л/1 - P2 cos2 a	, tan a
В = В V L—з  tan a' = -y= i-₽2 vr?
3.369
(a) We see that, E''B'= Е'ц • Е'ц + E'± • B'
_	(в1 - vxE
( f (£x + v*xB)-[ 1 c2
= E\\' -®n +
2 r-v-c
-» Е1-£1-(ЙВ)-(Гх£)/с2
Е| В|+~ ~
—► —► E. • В. - (y\ B. ) • (vxE. )/c2
 £| Я| ♦------~2--------
But,	AxBCxD= ACBD-ADBC,
so,	E' ‘В's Ец • By + E^ • B^-2~ = E • В
1-7
(b) E'2 - с2 B'2 - £'j| - c2 B'j| + £'2 - c2 B'l
407
vxE \
(el + v‘k^)2-c2\Bl- , I cr
-4-c4+~v
1~г
4-c2^+~v ’ 1_c2
= E^-c2B^ + -^j[E2-c2B2](1-^)= E2-c2B2,
i _—	\c)
c2
since, (vxA^)2» v2A2
E\ - с2 в\ + (Гх в! )2 - 4	EJ2
c
3370 In this case,E'B- 0, as the fields are mutually perpendicular. Also,
2
Е^-с2^- -20x108|—I is -ve.
ml
Thus, we can find a frame, in which E' = 0, and
/2	/	/	4	\ 2
В' = -y/c2B2-E2 = В у 1 - -£-=• = 0-20 V1 - I--T - 015 mT
c	c2B2	(3 x 108 x 2 x 10" j
3371 Suppose the chaige q moves in the positive direction of the x-axis of the frame K. Let us go over to the moving frame K', at whose origin the charge is at rest. We take the x and x' axes of the two frames to be coincident, and the у & у' axes, to be parallel.
In the K' frame, E = —~
4ле0 r'3
and this has the following components, E' -	E'- -J—
x 4ле0/3’ у 4ле0 r'3
Now let us go back to the frame K. At the moment, when the origins of the two frames coincide, we take t« 0. Then,
x = r cos 0 = x' у 1 - — , у = r sin 0 = у'
Also,
Ex - Ey = EJ / Vl-v2/c2
_ л t. ,2 r (1 - P sin 0)
From these equations, r = ——2-----------------
___2---------1_ o2x3/2 / / *	— .• 4neor3(1_₽2sin20)3/2pl	₽)	+
<H1-P2)
4 Jt e0 r3 (1 - p2 sin2 0)3/2
408
3.7 MOTION OF CHARGED PARTICLES IN ELECTRIC AND MAGNETIC FIELDS
3372 Let the electron leave the negative plate of the capacitor at time t = 0 a	„ dtp cp at
’	x dx' l Г
and, therefore, the acceleration of the	electron,
eE	eat	dv	eat
w= —=	—7	or,	— «	—г
m	ml	dt	ml
V	t
/,	ea f ,	1 ea 2
dv- —- I tdt, or, v — — —-t ml J	2 ml
о	0
(1)
But, from 5
1
/б TWZ2V or, r= -------
I ea J
ea?
6 ml
/ 1“
2 ml J 0
Putting the value of t in (1), 2	1
lea(6ml2\3 /9 ale\3 , v = — —71---- « I — — - 16 km/s.
2 ml I ea 1	[2 m
3.373
w =
The electric field inside the capacitor varies with time as, E= at.
Hence, electric force on the proton, F- eat and subsequently, acceleration of the proton, eat m
Now, if t is the time elapsed during the motion of the proton between the plates, then t« —, as no acceleration is effective in this direction. (Here v is velocity along the length
of the plate.)
dv±
From kinematics, —7- = w dt
so,
(as initially, the component of velocity in the direction, ± to plates, was zero.)
409
С еа t2 еа I2 or	v = । — — = ---------
х J т 2т 2m у2 о	II
Now,	tan а = — =
'll 2mvJ
1
eal2 •	(2eV\2
- ---------as v - --------- , from energy conservation.
± Him] o (2eV\i	'	'
2m I-----
I m j
al2 ^Г~т 4 *2 eV5
3.374 The equation of motion is, dv	dv	x
dt	dx	mx 0	'
Integrating
~ v2 - (Eqx - ~ ax2) = constant. Z m - Z
But initially v = 0 when x = 0, so “constant” = 0
Thus,	v2 = ^2. [£ x - 4 ar21
m I 0	2 I
2£0
Thus,v = 0, again for x = xm = ——
The corresponding acceleration is,
И ’m(£o-2£o)-- — \ /* '	' m
3.375 From the law of relativistic conservation of energy
WoC2	2
- — - eEx = mQc . Vl-(v^/?)	0
as the electron is at rest (v = 0 for x = 0) initially.
Thus clearly	T= eEx.
On the other handjYl^Cv/c2) = -----z-----
m0 c + eEx
v V(m0 c2 + e Ex)2 - m^ c4
c m0 c2 + eEx
С C (wo c2 + eJ^x) ct= I cdt= 1
or,
V(m0 c2 + eEx)2 - m^ c4
410
-у=^==« V(m0 с2 + eEr)2 - с4 + constant
V у - ти0 с
The “constant” = 0, at t« 0, for х « О,
So,	cr-	V(/ПдС2 + еЕх)2	с4.
Finally, using Т= еЕх,
сеЕ Го« у/т(Г+2т0с2) or,
3.37^ As before, Т = еЕх Now in linear motion, d mQv mQw
у/т(Г+2т0с2) f°“ eEc
m0
= (l-v2/?)372""
(T+moc2)3
----4-7---eE, m^c
eEm2c6 eE! T
So, w « ----------^-5-« — 1 +-----7
(T + m0 c2)3 wo I m0 c2
-3
3.377
The equations are, d(	1 П A d
<*IV1 -(v^/c2) I	dt
' mQvy
Vl - v2/c2
= eE
Hence,
vo
Vx
--========== constant = -7================
VT77?	vi-(v2/?)
Also, by energy conservation, 2	2
mQ C	mQC
—====== ^ . :=+eEy Vl-O^/c2) Vl-(^/c2)
2 v0 e0	m0 c
V = ------—, £n =
£0 + e^	Vl-(v^/c2)
mQ	e0 + e£y
Vl - (v^ / c2)	c2
(e0 + eEy) vy - c2 eE t + constant
“constant” = 0 as v^s 0 at 0.
Integrating again, 1	2	1 2	2
e0у + — eEy = — c Et + constant.
Dividing
Also,
Thus,
/
411
“constant” = 0, as у = 0, at t = О.
Thus,(се E г)2 - (eyE)2 + 2 е0 еЕу +
or,	ceEt= V(Eo + eEy)2-£o
or,	e0 + eEy = Veq + c2e2E2r2
Hence,
and
vo eo -	c2 e Et
Ve2 + c2e2E2/2 У ^2 + c2e2E2t2
tan 0 = -2- = -^-Vl-(v2/c2). vx m0 v0
3.378 From the figure,
sina =
d_ = dQB R mv ’
m v
As radius of the arc R = —— > where v is the qB
velocity of the particle, when it enteres into the field. From initial condition of the problem,
Hence, sin a =
and a = sin-1 \dB V I = 30°, on putting the values.
„2 mV n
—— = evB or, v = R
3.379 (a) For motion along a circle, the magnetic force acted on the particle, will provide the centripetal force, necessary for its circular motion.
eBR
m
and the period of revolution,T = — = --------= ——
co v eB
(b) Generally, = F
But	d	то*	4____________
U ’ Л	dt Vl-O^/c2)	Vl-(v2/c2) + (1 - (V2 / c2))
For transverse motion, v • v = 0 so, m0 v*	m0 v2
dt = Vl-(vW) = Vl-(v2/c2) r ’
m0 vJiT
3/2	2
412
Thus,	—7	= Bev or, . .	. = = ----
rVl-(v2/c2)	Vl-^/c2) m°C
v ______Ber____
c~ VtfW + ^c2
Finally,, T= ------= ^7в2е2г2 + /п2с2
v eB<T~M cBe
3.380 (a) As before,p « В qr.
(b) T = Vc2p2 + m2c4 = Vc2^2 92r2 + m2c4
v2
(c) w = — = —----------------—
r г Г1 + (m0c/Bgr)2l
using the result for v from the previous problem.
3.381 From (3.279), 2 л e
T= -z—(relativistic),	To= —z--------(nonrelativistic),
с eB	c eB
Here,	m0 c2 / Vl - v2/c2 « E
Thus,	6T-	(T~ K.E.)
c2eB
kt	6T T	„	2
Now,	- T) = ----у > so> T» T) m0 c
To moc
3.382 T= eV =^mv2
(The given potential difference is not large enough to cause significant deviations from the nonrelativistic formula).
Thus,
So,
Now, and
2 яг 2 Jim v± Be
Pitch
413
3383 The charged particles will traverse a helical trajectory and will be focussed on the axis after traversing a number of turms. Thus
2лт z 2лт
—— « (л +1) ——
qB2
I — - Л* vo
л + 1
So,
n B~i
b2
1 B2-Bi
Непсе,
2лт q(B2-BY)
vo
or,
I2 2qV I m
(W 1 (B2-^)2 (q/m)2
or,
j? = 8л2У m l2(B2-B^
3.384 Let us take the point A as the origin О and the axis of the solenoid as z-axis. At an arbitrary moment of time let us resolve the velocity of electron into its two rectangular components, along the axis and t0 ibe axis of solenoid. We know the magnetic force does no work, so the kinetic energy as well as the speed of the electron | v± | will remain constant in the x-y plane. Thus can change only its direction as shown in the Fig.. Гц will remain constant as it is parallel to B. Thus at Г = Г
vx = v± cos cor = v sin a cos cor,
vv  v. sin cor - v sin a sin cor
and
v = v cos a , where co = — z	m
As at r = 0, we have x = у = z = 0, so the motion law of the electron is.
v cos a r vsina . -------sm co r
co
v sin a z „ 4
-------(cos co r - 1) co
(The equation of the helix)
On the screen,
Z,
I so r® -----
vcosa
Then,
2 v2 sm2 a Л coZ -----z---- 1 - cos--------
co2 I v cos a
z =
У
2	, 2
2 v sin a . co
(til sm -------------
2 v cos a
mv .
= 2 —sma eB
leB sm -----------
2 mv cos a
414
3.385
Choose the wire along the z-axis, and the initial direction of the electron, along the x-axis. Then the magnetic field in*the x-z plane is along the у-axis and outside the wire it is,
By-В,-0, i£ у-0)
The motion must be confined to the x-z plane. Then the equations of motion are, d cfimvx~ -evzBy
d (mvz)	D
dt - +ev*By
Multiplying the first equation by vx and the second by vz and then adding, dvz
Vv“T“ + Vy — = 0 x dt z dt
+ v2 « vi. x Yz 0 ’
or,
Then,
say, or, v2- Vvg-v*
V* dk =
m
2	2 HQ J
vn - vv -—
0 x 2nx
vdv,
or,
Me dx 2лт x
x
-----Ш “
2 mi a
on using,vx = v0 , if x = a (i.e. initially).
Now,	vx = 0, when x = xm,
Integrating,
3.386
v/b .	, V-oIe
x = a e ° , where b = ------.
m	2mi
Inside the capacitor, the electric field follows a ~ law, and so the potential can be written as
_ V lnr/a -V 1 In ft/л ’ Inb/a r
Here r is the distance from the axis of the capacitor.
mv2 qV 1	2 QV
----  —x--------or mv « —*—*-
r In ft / ar r	In ft / a
On the other hand, mv - q В r in the magnetic field. V л q v	V
v = -z—;—r~ and = — = -у-з;--------------
В r In ft / a m Br B2 r2 In (ft / a)
so,
Also,
Thus,
415
3.387 The equations of motion are, dv	dvz
-qBv2,	qE and т~^~~ clvx^
These equations can be solved easily.
First,	v- — Г, тг—r'2
у m z 2m
Then,	v2 + v2 = constant » as before.
In fact, vx = v0 cos cor and v2 = v0 sin cor as one can check.
Integrating again and using x = z = 0, atr=O vo •	, voZl л
X = — sin cor, z = — (1 - cos СОГ) co	co
2л
Thus,	x- z = 0 for r = Г = n —
n co qE 2л 2 2л 2л2тЕи2 At that instant, v = -J— x ——— x n x ———  -----------~-----
2m qB/m qB I m
v„
Also,	tan an = ~, (y2 = 0 at this moment)
vy
= mvo mv0 QB 1 Bvo qEtn qE m 2 ли 2л£и*
3.388 The equation of the trajectory is, x = sin cor, z = ~ (1 _ 008 <°r), у =	r2 as before see (3.384).
Now on the screen x = Z, so coZ	. -1 coZ
sin cor = — or, vo At that moment, ?£ / . У» —	j SI 2 m co2 coZ . \ so,	= sm V	zr^“ v0	qE .	V0o . 2 and	z « — 2 sm — co	2 .	1	. -i <пГ « /tan— sin —  2	*o For small (t 2mE 1 2mE 2 or,	у - о - . z	cor - sm — vo ,42 -1 coZ \ n 	 v° / • \hqB2y “ Sm V Em - Ztany 2mE zj " /2 is a parabola.
416
3389
In crossed field,
г	E
eE = evB, so v = -
В
I	E
Then^F = force exerted on the plate = - x m — * €	В
mlE
3390
When the electric field is switched off, the path followed by the particle will be helical, and pitch, А/ = T, (where v is the velocity of the particle, parallel to Д and T, the time period of revolution.)
= v cos (90 - <p) T = v sin ф T f	2л'
as T ® — k	4s
Now, when both the fields were present, qE = qvB sin (90 - ф), as no net force was effective on the system.
= vsinqp
(1)
or,
From (1) and (2),
E v = ------
В cos ф
AI £ 2 лт , A/ =	—— tan op « 6 cm.
В qB
(2)
3391
(1)
When there is no deviation, ,	,
-qE~ q (v*x В )
or, in scalar from, E « vB (as v ± В ) or, v = — В
Now, when the magnetic field is switched on, let the deviation in the field be x. Then,
x= L(zaB\t2 21 m I
where t is the time required to pass through this region.
a t- -v
also,
1 Я a2 В2 |	2 m E
For the region where the field is absent, velocity in upward direction
I m J m
Thus
(2)
(3)
Now,
m
From (2) and (4),
or,
q aB2b -	,
-3---— when t
m E
v“ E
aB2b
. loa2B2 4
Ar-—-2—— = ~2-2 m E m E
q 2E Ax m“ ^^(а + гб)
(4)
417
3.392 (a) The equation of motion is, d2r* -> _> -» m ^2 = q(E + v*B)
Now,
i j к x у z
О В
iBу ~jВх
vxB
0
<4	qE qB dvz	_
_ 21— _ -2— v	ancj _ Q
dt	m	m x dt
So, the equation becomes, dvx qBvy dt m
Here, vx - x, vy « у, vz - z. The last equation is easy to integrate: vz « constant « 0, since vz is zero initially. Thus integrating again, z = constant = 0,
and motion is confined to the x - у plane. We now multiply the second equation by i and add to the first equation.
vx+ivy
we get the equation,
d± . E . t	qB
dt В ъ	m
This equation after being multiplied by e,ft)/can be rewritten as, at	d
and integrated at once to give, fc	С о "1~1a
*~B + C
where C and a are two real constants. Taking real and imaginary parts. £
vx = ~ + C cos (co t + a) and v = - C sin (cor + a) В	у	~
Since = 0, when t = 0, we can take a = 0, then vx = 0 at t = 0 gives, C = -and we get,
bo
E	E
vx = 2? (1 ” 008 and Vy = — sin cot
Integrating again and using x = у - 0, at t« 0, we get z ч E / sin cor\ . ч E z.	4
*0) = д Г_—77”’	77R^-C0St0^
D I CO J	СОЛ
This is the equation of a cycloid.
(b) The velocity is zero, when cor - 2 n я. We see that
!E\2
v2 - vj + v2 - I — j (2-2 cos cor) y I" J
418
ds 2E
V= dt" В
or,
. (tit sin 2
The quantity inside the modulus is positive for 0 < (tit < 2 я. Thus we can drop the modulus and write for the distance traversed between two successive zeroes of velocity.
c 4E / coA S =	- cos —
coB 2 I
Putting
cof = 2 л, we get
8E 8 mE
* coB “ q B2
(c) The drift velocity is in the x-direction and has the magnitude,
j(l - cos
В *
3.393
p07
When a current I flows along the axis, a magnetic field Вф = —— is set up where 2	2	2
p - x + у . In terms of components, p0/y	цп7х
Bx------5—7, В = -5-7 and В ~ 0
x 2 л p2 y 2 n p2
Suppose a p.d. V is set up between the inner cathode and the outer anode. This means a potential function of the form T7 In p/b	.
v'ina/b’a>p>b’
as one can check by solving Laplace equation. The electric field corresponding to this is, £ = _.........
x p2 In a I b ’ y p2 In a I b
The equations of motion are, \e\Vz И M . p2 In a I b 2 я p2 |e|Vy	•
—1——— +----------у z
p2 In a / b 2 я p2
d — mv~ dt x
d dtmVy~
0.
and
d	I I Vo1 ( .	• ,
—	-|e|------i<xx+yy)
2 я p
(- | e |) is the charge on the electron.
Integrating the last equation,
M d .
-— — In p 2 л dt r
i i цо71	/
mv « -|e| —lnp/a« mz.
2 я
419
since vz = 0 where p - a. We now substitute this z in the other two equations to get d (1	2 1	2\
T + ~mv\ dt\2	x 2	yl
2
Integrating and using v « 0, at p = b, we get,
1
2
LiLLi £ J_ а П b 2m ln6
The RHS must be positive, for all a > p > b. The condition for this is,
I I ✓	2
1	a
2 m 2л b
3.394 This differs from the previous problem in (a *-*b) and the magnetic field is along the z-direction. Thus B=B = 0, B= В
Assuming as usual the chaige of the electron to be -1 e |, we write the equation of motion d |e|Vx	d |e|Vy .
dtmv*‘ ~Гь-^Ву' dtmvy’ p In-	p in-
and	— mv = 0 «> z« 0
dt z
The motion is confined to the plane z = 0. Eliminating В from the first two equations,
v2\	**+уу
dt |2	) lnb/а p2
or,
^mv2= | e | V
In p/a In b/a
so, as expected, since magnetic forces do not work,
v =
, at p =
b.
420
On the other hand, eliminating V, we also get, ^m(xvy-yvx)~ felB(xx + yy)
i.e.
At
. ч I € IВ 2 (xVy-yvJ- 2w P +conslant
The constant is easily evaluated, since v is zero at p - a. Thus,
(XV -yvx) - Ц^( P2 - a2) > 0
b, (xVy-yvJ* vb
Thus,
Vbb^-(.b2-a2) 2m
or,
2 mb
«,2	„2
о — a
1
x 1—Г
or,
2b л/ЪпВ b2-a2N |e|
fl* dt
with
3395 The equations are as in 3.392.
dvy <1E	, qB . dvz
v , —- ------cos cot - -1— v, and — -
m y dt m	m * dt
co -	| - vx + iv^ we get,
<&	Em
-^ = i —co cos ent-ico S dt. В	*
etw>,
i^(e2iat+l) at	2d
or multiplying by
or integrating,	^eta,t• ^e2,at+ ^imt
or,	Ц~^(ei^a, + 2i<^aei^м) + Cei^tt,
Ad
Em
since	^-Oatr«O, C«- —.
Ad
Em	Em	imt
Thus,	i—sin<or + i—cote
2d	2d
Em	Em Em
or>	vx~ zz’cotsinent and vv» — sin cot+ —cot cos cot
а/i	у ЛН
Integrating again,
x - -—x (sin cot - cot cos cot), у =	1 sin cot.
2<o2	2co
421
3396
3.397
qEm
where a - -----, and we have used x - у - 0, at t - 0.
m	7
The trajectory is an unwinding spiral.
We know that for a charged particle (proton) in a magnetic field, mv2 ----------------------------------- В ev or mv = Ber r
But,
Thus
eB co - — m
—	1	2	1	2 2
£= — mv - —тю r.
2	2
ДЕ = mco2 r Ar = 4 л2 v2 mr &r
- 2 eV, where V is the effective acceleration voltage, across the
So, On the other hand ДЕ Dees, there being two crossings per revolution. So, Vs 2л2V2 mr br/e
2
. .	-1- WV n
(a) From--------= Bev, or,
mv = Ber
and
T =
(Ber)2 2m
- ^mv2 - 12 MeV 2
(b)
„ 2л
From — CO
2л r v
3.398
1 Гт — X/^~ - 15 MHz nr 2m
The total time of acceleration is,
1 t= -z—'n, 2v
where n is the number of passages of the Dees.
B2e2r2 T= neV= ? - * -2m
we
(a)
get,
But,
or,
f________v-
mi° 2 nr
B2 er2
n~ 2mV
л B2 er2 it В г2 л2 mv r2
f	— x —-— =	= -----— = 30 |X s
eB I m 2mV 2V eV
(b) The distance covered is, s = vn ’ ^7
So,
But,
4
2eV r---- УП ,
m
So, s
eV 2 3/2 2mv2 3
422
But,
Thus.
B2e2r2 2n2mv2r2
П~ 2eVm ~ eV
4 л3 v2 mr2	0 л
—3eV~ ’ 124km
3.399
2 nr	n
In the nth orbit, ------ = n To= -• We
Vn	V
vn« c. Also cp- cBern.
ignore the rest mass of the electron and write
Thus,
2nW Bee2
n
3.400 The basic condition is the relativistic equation,
= Bqv, or, mv - -====== = Bqr. r	Vl-v2/?
Or calling,
Bq co = -л-m
we get,
co =
<o0____
----ГТ
co0r
+ „2
Bq
wo= ZT 7И0
is the radius of the instantaneous orbit. The time of acceleration is,
N
N
у -L.y i
2v„ %
n  1
N
V
Zj qBc2'
1
N is the number of crossing of either Dee.
But, Wrt =
2 mQc +
nAW
2
, there being two crossings of the Dees per revolution.
V' 717ПОС2 V' JtAW_
So>	qBc2 + 2qBc2
Kr 71	^(^+l)7tAJУ KT2 JtAW
— +----------— e -------
«0	4 qBc2 4 qBc2
(N>> 1)
Also,
_	Vn c d* AW
r~	л dN* 2qBc
423
3.401
3.402
3.403
Непсе finally,
_______________________
V. д2в2 &w2 n2
1 +	2 2 X д 2 n2 y2
w0 c 4 q В c
_____^0___________coo
V1+(aw)2x4<^7~ vr7"’
4m2c4 nW*
qB&W ах — ГТ itmQc
When the magnetic field is being set up in the solenoid, and electric field will be induced in it, this will accelerate the charged particle. If В is the rate, at which the magnetic field is increasing, then.
n •	1
nrB* 2nrE or E = —rB
dv 1 •	qBr
тЛ-2гВ‘1’ ""Ь
After the field is set up, the particle will execute a circular motion of radius p, where
mv = В q p, or p = — r
The increment in energy per revolution is e <t>, so the number of revolutions is,
W
еФ
Thus,
The distance traversed is, s = 2 itrN On the one hand,
dt
e t/Ф
2яг dt
e d C
2яг dt J о
On the other ,
so,
Hence,
So,
p = В (r) er, r = constant.
= er ~ В (r)  er В (r) dt dt '
eri^-
B<r>‘H<B>

This equations is most easily satisfied by taking В (rQ) -	< В >.
ro
1 If	2
3.404 The condition, В (r0) e — < В > = —J B- 2яг dr/nr0 о
424
or,	В (r, J  Л f Brdr
'И
This gives r0.
In the present case, ro B0-at%- -^£(В-ar^rdr- Ув0-^аг% ro о	\	>
or,
or r0-
3.405 The induced electric field (or eddy current field) is given by, r
2лг at J
о
Hence,
dE dr
2nr'B(r’)dr' +
dB(r) dt
3.406
Id . dB(r) - ~- — <B> + — 2dt	dt
This vanishes for r « r0 by the betatron condition, where r0 is the radius of die equilibrium orbit
From the betatron condition,
1 d D dB . . 2 dt	dt v °7
В
d 2B Thus,	=-<B>-=£-
dt	bt
,	</Ф id<B > 2nr2B
and	— - nr*—-—- —-—
dt	dt bi
So, energy increment per revolution is, d Ф	2 л r2eB
e dt “ bi
3.407 (a) Even in the relatjvistic case, we know that: p = Ber
Thus, W = Vc2/»2 + OTqC4 - m0c2 = m0 c2 ^V1 + (Ber I	-1)
(b) The distance traversed is,
W	W	Wbt
2nr—57“ 2лг----5-------- ——,
еФ	глг’еВ/Дг	Ber
on using the result of the previous problem.