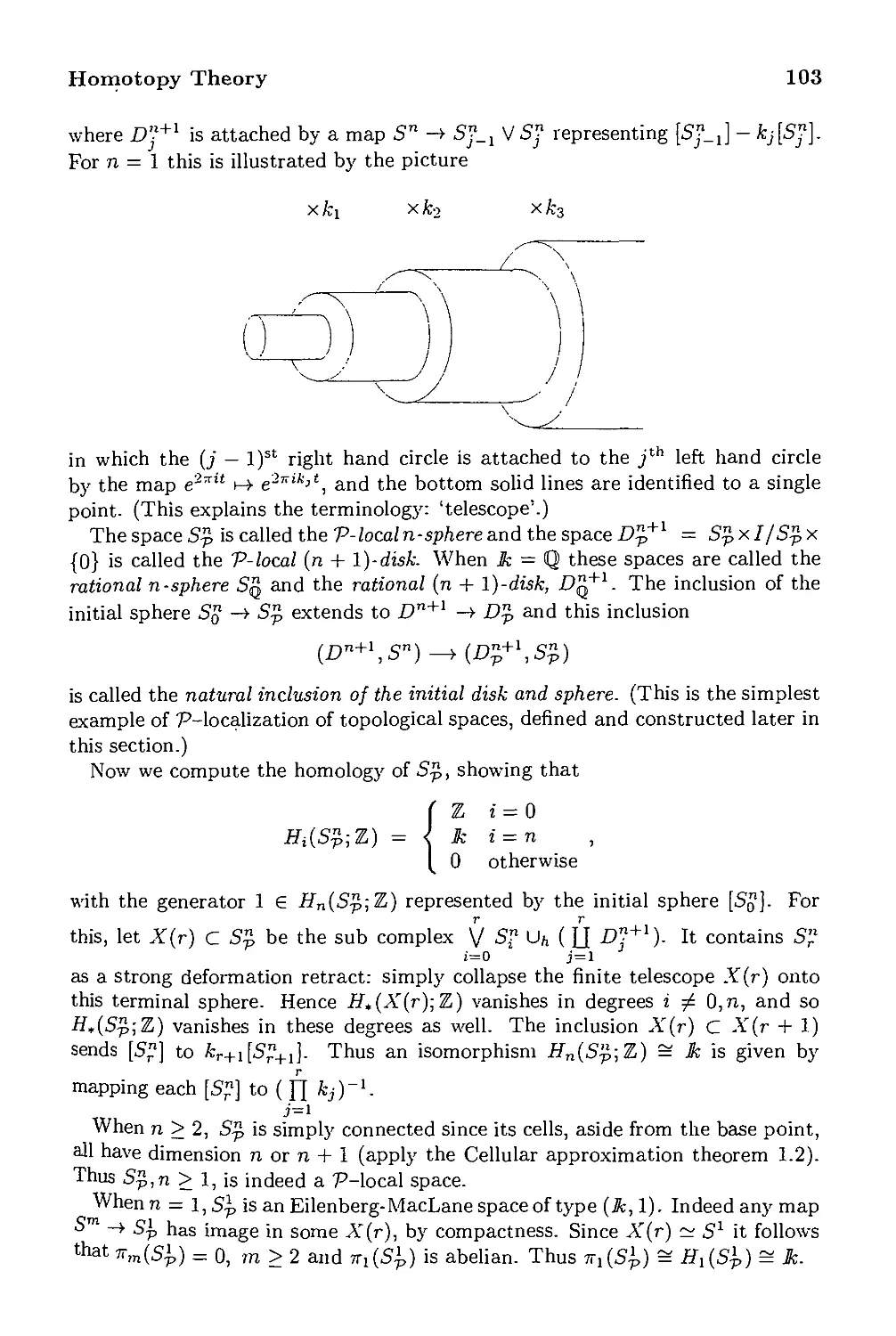
Автор: Félix Y. Halperin S. Thomas J.-C.
Теги: rational homotopy theory homotopy
ISBN: 0-387-95068-0
Год: 2001
Текст
Graduate Texts in Mathematics
1 Takeuti/Zaring. Introduction to
Axiomatic Set Theory. 2nd ed.
2 Oxtoby. Measure and Category. 2nd ed.
3 Schaefer. Topological Vector Spaces.
2nd ed.
4 Hilton/Stammbach. A Course in
Homological Algebra. 2nd ed.
5 Mac Lane. Categories for the Working
Mathematician. 2nd ed.
6 Hughes/Piper. Projective Planes.
7 Serre. A Course in Arithmetic.
8 Takeuti/Zaring. Axiomatic Set Theory.
9 Humphreys, introduction to Lie Algebras
and Representation Theory.
10 Cohen. A Course in Simple Homotopy
Theory.
11 Conway. Functions of One Complex
Variable I. 2nd ed.
12 Beals. Advanced Mathematical Analysis.
13 Anderson/Fuller. Rings and Categories
of Modules. 2nd ed.
14 Golubitsky/Guillemin. Stable Mappings
and Their Singularities.
15 Berberian. Lectures in Functional
Analysis and Operator Theory.
16 Winter. The Structure of Fields.
17 Rosenblatt. Random Processes. 2nd ed.
18 Halmos. Measure Theory.
19 Halmos. A Hubert Space Problem Book.
2nd ed.
20 Husemoller. Fibre Bundles. 3rd ed.
21 Humphreys. Linear Algebraic Groups.
22 Barnes/Mack. An Algebraic Introduction
to Mathematical Logic.
23 Greub. Linear Algebra. 4th ed.
24 Holmes. Geometric Functional Analysis
and Its Applications.
25 Hewitt/Stromberg. Real and Abstract
Analysis.
26 Manes. Algebraic Theories.
27 Kelley. General Topology.
28 Zariski/Samuel. Commutative Algebra.
Vol.1.
29 Zariskj/Samuel. Commutative Algebra.
Vol.11.
30 Jacobson. Lectures in Abstract Algebra I.
Basic Concepts.
31 Jacobson. Lectures in Abstract Algebra II.
Linear Algebra.
32 Jacobson. Lectures in Abstract Algebra
III. Theory of Fields and Galois Theory.
33 Hirsch. Differential Topology.
34 Spitzer. Principles of Random Walk.
2nd ed.
35 Alexander/Wermer. Several Complex
Variables and Banach Algebras. 3rd ed.
3 6 Kelley/Namioka et al. Linear Topologi cal
Spaces.
37 Monk. Mathematical Logic.
38 Grauert/Fritzsche. Several Complex
Variables.
39 Arveson. An Invitation to C*-Algebras.
40 Kemeny/Snell/Knapp. Denumerable
Markov Chains. 2nd ed.
41 Apostol. Modular Functions and
Dirichlet Series in Number Theory.
2nd ed.
42 Serre. Linear Representations of Finite
Groups.
43 Gillman/Jerjson. Rings of Continuous
Functions.
44 Kendig. Elementary Algebraic Geometry.
45 Loeve. Probability Theory I. 4th ed.
46 Loeve. Probability Theory II. 4th ed.
47 Moise. Geometric Topology in
Dimensions 2 and 3.
48 Sachs/Wu. General Relativity for
Mathematicians.
49 Gruenberg/Weir. Linear Geometry.
2nd ed.
50 Edwards. Fermat's Last Theorem.
51 Kxingenberg A Course in Differential
Geometry.
52 Hartshorne. Algebraic Geometry.
53 M.wiN. A Course in Mathematical Logic.
54 Graver/Watkjns. Combinatorics with
Emphasis on the Theory of Graphs.
55 Brown/Pearcy. Introduction to Operator
Theory I: Elements of Functional Analysis.
56 Massey. Algebraic Topology: An
Introduction.
57 Crowell/Fox. Introduction to Knot
Theory.
58 Koblitz. p-adic Numbers, p-adic
Analysis, and Zeta-Functions. 2nd ed.
59 Lang. Cyclotomic Fields.
60 Arnold. Mathematical Methods in
Classical Mechanics. 2nd ed.
61 Whttehead. Elements of Homotopy
Theory.
62 Kargapolov/Merlzjakov. Fundamentals
of the Theory of Groups.
63 Bollobas. Graph Theory.
64 Edwards. Fourier Series. Vol. I. 2nd ed.
(continued after index)
Yves Felix
Stephen Halperin
Jean-Claude Thomas
Rational Homotopy Theory
Yves Felix
Institut Mathematiques
Universite de Louvain La Neuve
2 chemin du Cyclotron
Louvain-la-Neuve, B-1348
Belgium
Jean-Claude Thomas
Faculte des Sciences
Universite d'Angers
2 bd Lavoisier
Angers 49045
France
Editorial Board
S. Axler
Mathematics Department
San Francisco State
University
San Francisco, CA 94132
USA
Stephen Halperin
College of Computer, Mathematical,
and Physical Science
University of Maryland
3400 A.V. Williams Building
College Park, MD 20742-3281
USA
F.W. Gehring
Mathematics Department
East Hall
University of Michigan
Ann Arbor, MI 48109
USA
K.A. Ribet
Mathematics Department
University of California
at Berkeley
Berkeley, CA 94720-3840
USA
Mathematics Subject Classification B000): 55-01, 55P62
Library of Congress Cataloging-in-Publication Data
Felix, Y. (Yves)
Rational homotopy theory / Yves Felix, Stephen Halperin, Jean-Claude Thomas
p. cm. — (Graduate texts in mathematics ; 205)
Includes bibliographical references and index.
ISBN 0-387-95068-0 (alk. paper)
1. Homotopy theory I. Halperin, Stephen. II. Thomas, J-C. (Jean-Claude) III. Title.
IV. Series.
QA612.7 .F46 2000
514'24—dc21 00-041913
Printed on acid-free paper.
© 2001 Springer-Verlag New York, Inc.
All rights reserved. This work may not be translated or copied in whole or in part without the
written permission of the publisher (Springer-Verlag New York, Inc., 175 Fifth Avenue, New York,
NY 10010, USA), except for brief excerpts in connection with reviews or scholarly analysis. Use
in connection with any form of information storage and retrieval, electronic adaptation, computer
software, or by similar or dissimilar methodology now known or hereafter developed is forbidden.
The use of general descriptive names, trade names, trademarks, etf., in this publication, even if the
former are not especially identified, is not to be taken as a sign that such names, as understood by
the Trade Marks and Merchandise Marks Act, may accordingly be used freely by anyone.
Production managed by Timothy Taylor; manufacturing supervised by Jacqui Ashri.
Camera-ready copy prepared from the authors' PDF files.
Printed and bound by R.R. Donnelley and Sons, Harrisonburg, VA.
Printed in the United States of America.
987654321
ISBN 0-387-95068-0 SPIN 10770738
- · -"-?.. m»„, YnrW Rerlin Heidelberg
to
Agnes
Danielle
Janet
Introduction
Homotopy theory is the study of the invariants and properties of topological
spaces X and continuous maps / that depend only on the homotopy type of the
space and the homotopy class of the map. (We recall that two continuous maps
/, g : X —> Y are homotopic (/ ~ g) if there is a continuous map F : Xxl -,—> Y
such that F(x,0) = f(x) and F(x, 1) = g(x). Two topological spaces X and ?
have the same homotopy type if there are continuous maps X ( ) Y such that
9
fg ~ idy and gf ~ ???.) The classical examples of such invariants are the
singular homology groups Hi(X) and the homotopy groups ??(?"), the latter
consisting of the homotopy classes of maps (Sn,*) —>· (X,x0). Invariants such
as these play an essential role in the geometric and analytic behavior of spaces
and maps.
The groups H{(X) and ??(?), ? > 2, are abelian and hence can be rational-
ized to the vector spaces Hi(X;Q) and ??(?) ® Q. Rational homotopy theory
begins with the discovery by Sullivan in the 1960's of an underlying geomet-
ric construction: simply connected topological spaces and continuous maps be-
tween them can themselves be rationalized to topological spaces Xq and to maps
/q : Xq —»· Yq, such that H.(XQ) = H*(X;Q) and n,(XQ) = ?*(?) ® Q. The
rational homotopy type of a CW complex X is the homotopy type of Xq and the
rational homotopy class of / : X —>· Y is the homotopy class of /q : Xq —»· 1q,
and rational homotopy theory is then the study of properties that depend only
on the rational homotopy type of a space or the rational homotopy class of a
map.
Rational homotopy theory has the disadvantage of discarding a considerable
amount of information. For example, the homotopy groups of the sphere S2
are non-zero in infinitely many degrees whereas its rational homotopy groups
vanish in all degrees above 3. By contrast, rational homotopy theory has the
advantage of being remarkably computational. For example, there is not even a
conjectural description of all the homotopy groups of any simply connected finite
CW complex, whereas for many of these the rational groups can be explicitly
determined. And while rational homotopy theory is indeed simpler than ordinary
homotopy theory, it is exactly this simplicity that makes it possible to address
(if not always to solve) a number of fundamental questions.
This is illustrated by two early successes:
• (Vigue-Sullivan [152]) // M is a simply connected compact riemannian
manifold whose rational cohomology algebra requires at least two generators
then its free loop space has unbounded homology and hence (Gromoll-Meyer
[73],) M has infinitely many geometrically distinct closed geodesies.
• (Allday-Halperin [3]) // an r torus acts freely on a homogeneous space G/H
(G and H compact Lie groups) then
? <rankG-rankii ,
viii Introduction
as well as by the list of open problems in the final section of this monograph.
The computational power of rational homotopy theory is due to the discovery
by Quillen [135] and by Sullivan [144] of an explicit algebraic formulation. In
each case the rational homotopy type of a topological space is the same as the
isomorphism class of its algebraic model and the rational homotopy type of a
continuous map is the same as the algebraic homotopy class of the correspond-
ing morphism between models. These models make the rational homology and
homotopy of a space transparent. They also (in principle, always, and in prac-
tice, sometimes) enable the calculation of other homotopy invariants such as the
cup product in cohomology, the Whitehead product in homotopy and rational
Lusternik-Schnirelmann category.
In its initial phase research in rational homotopy theory focused on the identi-
fication of rational homotopy invariants in terms of these models. These included
the homotopy Lie algebra (the translation of the Whitehead product to the homo-
topy groups of the loop space ??" under the isomorphism tt*+i(X) = ?*(?.?)).
LS category and cone length.
Since then, however, work has concentrated on the properties of these in-
variants, and has uncovered some truly remarkable, and previously unsuspected
phenomena. For example
• If X is an ?-dimensional simply connected finite CW complex, then either
its rational homotopy groups vanish in degrees > 2n, or else they grow
exponentially.
• Moreover, in the second case any interval (k, k + n) contains an integer i
such that iti(X) 0 Q ? 0.
• Again in the second case the sum of all the solvable ideals in the homotopy
Lie algebra is a finite dimensional ideal R, and
dim ?even 5; cat -^Q ·
• Again in the second case for all elements a G iieVf,n(Q.X) ® <Q> of sufficiently
high degree there is some ? G ?,.(??')®(?! such that the iterated Lie brackets
[a, [a,..., [?, ?] ¦ ¦ · ]] are all non-zero.
• Finally, rational LS category satisfies the product formula
cat(X<Q ? Yq) = catXQ -f catYq ,
in sharp contrast with what happens in the 'non-rational' case.
The first bullet divides all simply connected finite CW complexes X into two
groups: the rationally elliptic spaces whose rational homotopy is finite dimen-
sional, and the rationally hyperbolic spaces whose rational homotopy grows ex-
ponentially. Moreover, because H*(fiX;Q) is the universal enveloping algebra
¦ v . —j„j T .„ „1n.oKra Tiv _ ?jftX") ®Q, it follows from the first two bullets
Rational Homotopy Theory ix
that whether X is rationally elliptic or rationally hyperbolic can be determined
from the numbers b{ = dim Hi(flX;Q), 1 < i < 3n — 3, where ? = dim .Y.
Rationally elliptic spaces include Lie groups, homogeneous spaces, manifolds
supporting a codimension one action and Dupin hypersurfaces (for the last two
see [77]). However, the 'generic' finite CW complex is rationally hyperbolic.
The theory of Sullivan replaces spaces with algebraic models, and it is exten-
sive calculations and experimentation with these models that has led to much of
the progress summarized in these results. More recently the fundamental article
of Anick [11] has made impossible to extend these techniques for finite CW com-
plexes to coefficients ? (-^-,..., ?- ) with only finitely many primes invested, and
thereby to obtain analogous results for H*(UX; Wp) for large primes p. Moreover,
the rational results originally obtained via Sullivan models often suggest possible
extensions beyond the rational realm. An example is the 'depth theorem' origi-
nally proved in [54] via Sullivan models and established in this monograph (§35)
topologically for any coefficients. This extension makes it possible to generalize
many of the results on loop space homology to completely arbitrary coefficients.
However, for reasons of space and simplicity, in this monograph we have re-
stricted ourselves to rational homotopy theory itself. Thus our monograph has
three main objectives:
• To provide a coherent, self-contained, reasonably complete and usable de-
scription of the tools and techniques of rational homotopy theory.
• To provide an account of many of the main structural theorems with proofs
that are often new and/or considerably simplified from the original versions
in the literature.
• To illustrate both the use of the technology, and the consequences of the
theorems in a rich variety of examples.
We have written this monograph for graduate students who have already en-
countered the fundamental group and singular homology, although our hope is
that the results described will be accessible to interested mathematicians in other
parts of the subject and that our rational homotopy colleagues may also find it
useful. To help keep the text more accessible we have adopted a number of
simplifying strategies:
- coefficients are usually restricted to fields k of characteristic zero.
- topological spaces are usually restricted to be simply connected.
- Sullivan models for spaces (and their properties) are derived first and only-
then extended to the more general case of fibrations, rather than being
deduced from the latter as a special case.
- complex diagrams and proofs by diagram chase are almost always avoided.
x Introduction
Of course this has meant, in particular, that theorems and technology are not
always established in the greatest possible generality, but the resulting saving in
technical complexity is considerable.
It should also be emphasized that this is a monograph about topological spaces.
This is important, because the models themselves at the core of the subject are
strictly algebraic and indeed we have been careful to define them and establish
their properties in purely algebraic terms. The reader who needs the machin-
ery for application in other contexts (for instance local commutative algebra)
will find it presented here. However the examples and applications throughout
are drawn largely from topology, and we have not hesitated to use geometric
constructions and techniques when this seemed a simpler and more intuitive
approach.
The algebraic models are, however, at the heart of the material we are pre-
senting. They axe all graded objects with a differential as well as an algebraic
structure (algebra, Lie algebra, module, ... ). and this reflects an understanding
that emerged during the 1960!s. Previously objects with a differential had often
been thought of as merely a mechanism to compute homology; we now know
that they carry a homotopy theory which is much richer than the homology.
For example, if X is a simply connected CW complex of finite type then the
work of Adams [1] shows that the homotopy type of the cochain algebra C*(X)
is sufficient to calculate the loop space homology H„(UX) which, on the other
hand, cannot be computed from the cohomology algebra H*(X). This algebraic
homotopy theory is introduced in [134] and studied extensively in [20].
In this monograph there are three differential graded categories that are im-
portant:
(i) modules over a differential graded algebra (dga), (R,d).
(ii) commutative cochain algebras,
(iii) differential graded Lie algebras (dgl's).
In each case both the algebraic structure and the differential carry information,
and in each case there is a fundamental modelling construction which associates
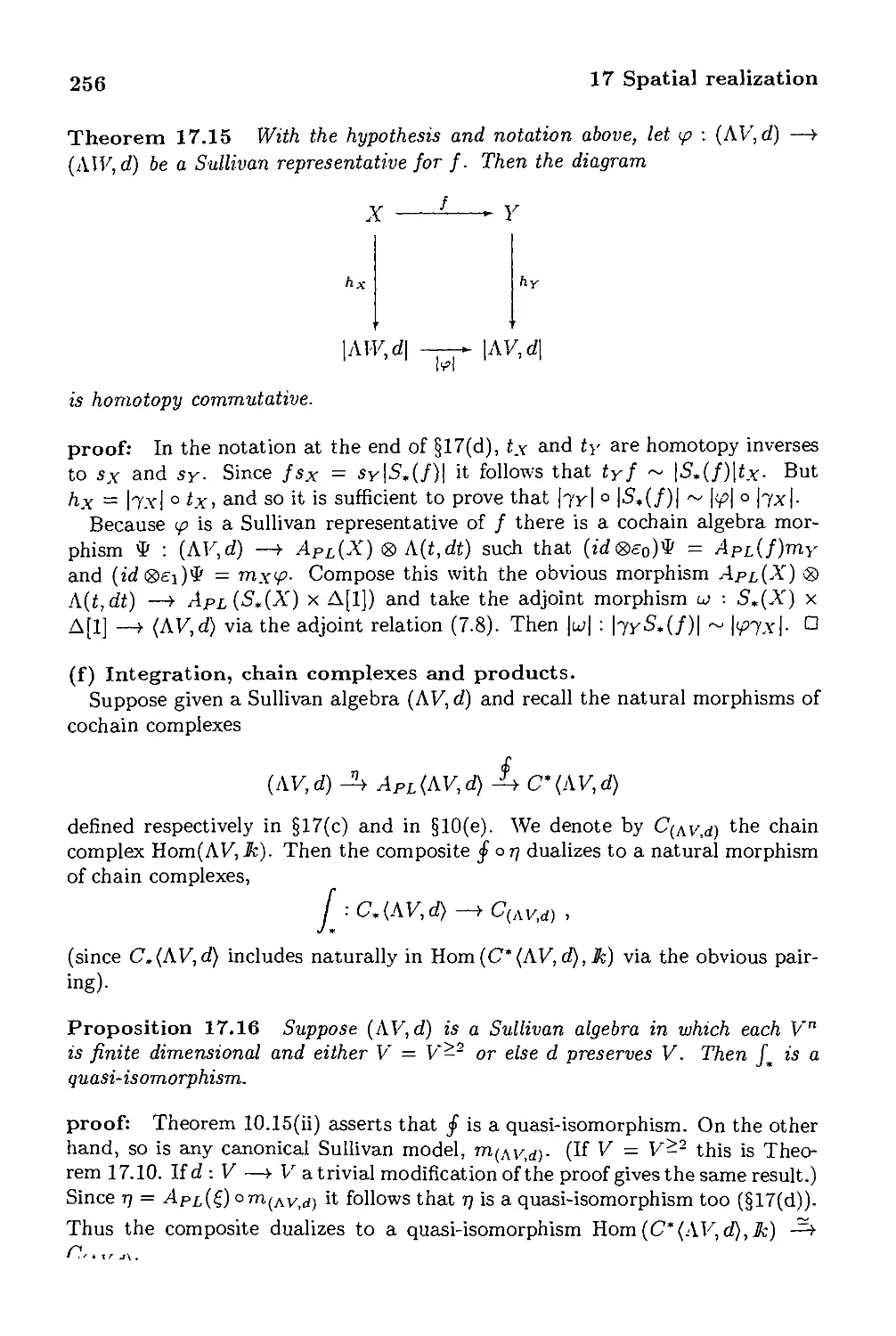
to an object A in the category a morphism
? : M —> A
such that ?(?) is an isomorphism (? is called a quasi-isomorphism) and such
that the algebraic structure in M is, in some sense "free".
These models (the cofibrant objects of [134]) are the exact analogue of a free
resolution of an arbitrary module over a ring. In our three cases above we find,
respectively:
(i) A semi-free resolution of a module over (R, d) which is, in particular a
m"in]pv of free A-modules.
Rational Homotopy Theory xi
(ii) A Sullivan model of a commutative cochain algebra which is a quasi-
isomorphism from a commutative cochain algebra that, in particular, is
free as a commutative graded algebra. (These cochain algebras are called
Sullivan algebras.)
(iii) A free Lie model of a dgl, which is a quasi-isomorphism from a dgl that is
free as a graded Lie algebra.
These models are the main algebraic tools of the subject.
The combination of this technology with its application to topological spaces
constitutes a formidable body of material. To assist the reader in dealing with
this we have divided the monograph into forty sections grouped into six Parts.
Each section presents a single aspect of the subject organized into a number
of distinct topics, and described in an introduction at the start of the section.
The table of contents lists both the titles of the sections and of the individual
topics within them. Reading through the table of contents and scanning the
introductions to the sections should give the reader an excellent idea of the
contents.
Here we present an overview of the six Parts, indicating some of the highlights
and the role of each Part within the book.
Part I: Homotopy Theory, Resolutions for Fibrations and P-local
Spaces.
This Part is a self-contained short course in homotopy theory. In particular,
§0 is merely a summary of definitions and notation from general topology, while
§3 is the analogue for (graded) algebra. The text proper begins with the basic
geometric objects, CW complexes and fibrations in §1 and §2, and culminates
with the rationalization in §9 of a topological space. Since CW complexes and
fibrations are often absent from an introductory course in algebraic topology we
present their basic properties for the convenience of the reader. In particular,
we construct a CW model for any topological space and establish Whitehead's
homotopy lifting theorem, since this is the exact geometric analogue, and the
motivating example, for the algebraic models referred to above.
Then, in §6, we introduce the first of these algebraic models: the semifree
resolution of a module over a differential graded algebra. These resolutions are
of key importance throughout the text. Now modules over a dga arise naturally
in topology in at least two contexts:
• /// : X —)¦ Y is a continuous map then the singular cochain algebra C*(X)
is a module over C*(Y) via C*(f).
• If ? ? G —)¦ X is the action of a topological monoid then the singular
chains Ct(X) are a module over the chain algebra C*(G).
In §7 we consider the first case when / is a fibration, and use a semifree
resolution to compute the cohomology of the fibre (when Y is simply connected
xii Introduction
with homology of finite type). In §8 we consider the second case when the action
is that of a principal G-fibration X —> Y and use a semifree resolution to
compute H*(Y). Both these results are due essentially to J.C. Moore.
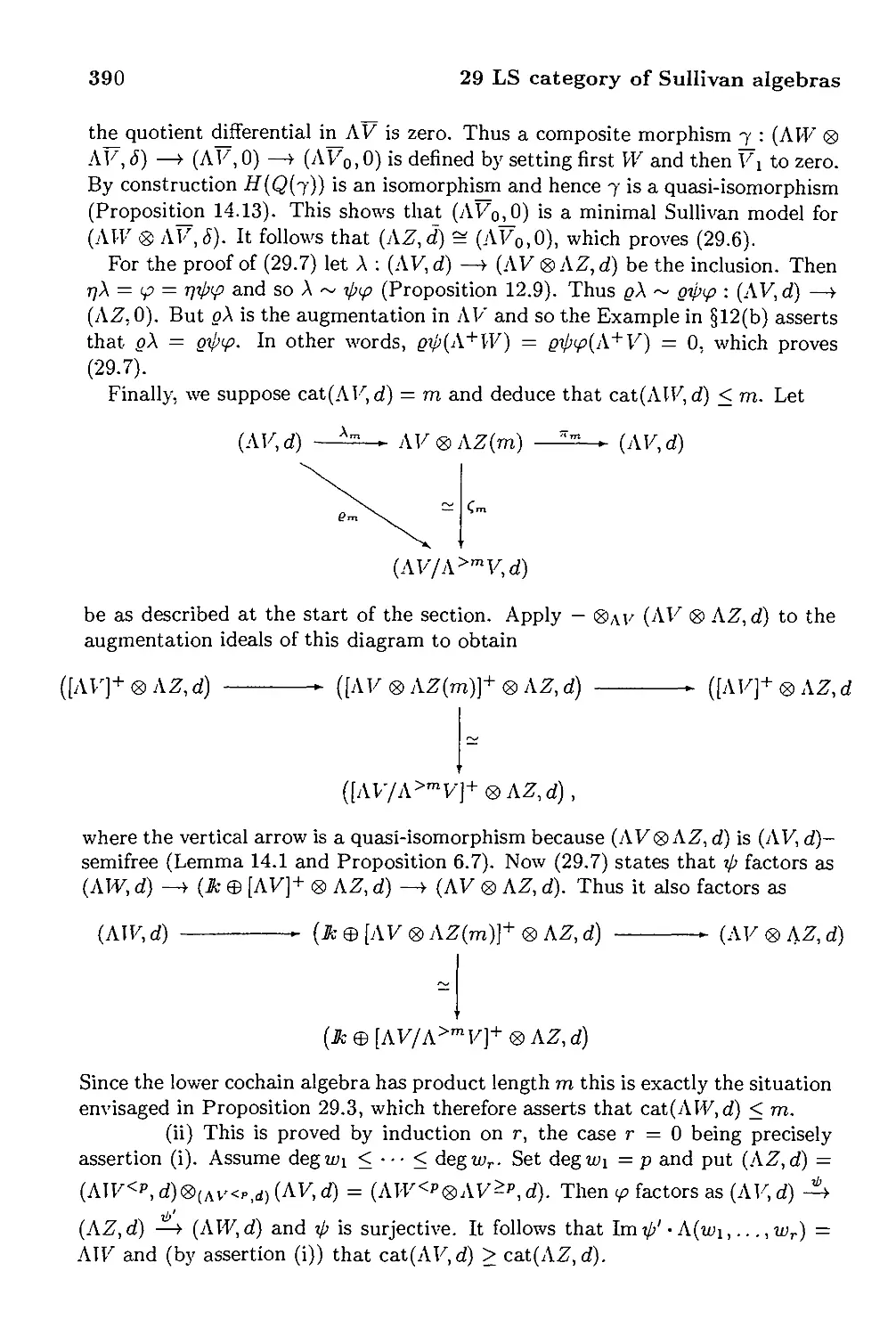
The second result turns out to give an easy, fast and spectral-sequence-free
proof of the Whitehead-Serre theorem that for a continuous map / : X —> Y
between simply connected spaces and for Jk C Q, Ht (/; Ik) is an isomorphism
if and only if 7r»(/) (g> h is an isomorphism. We have therefore included this as
an interesting application, especially as the theorem itself is fundamental to the
rationalization of spaces constructed in §9.
Aside from these results it is in Part I that we establish the notation and
conventions that will be used throughout (particularly in §0—§5) and state the
theorems in homotopy theory we will need to quote. Since it turned out that
with the definitions and statements in place the proofs could also be included
at very little additional cost in space, we indulged ourselves (and perhaps the
reader) and included these as well.
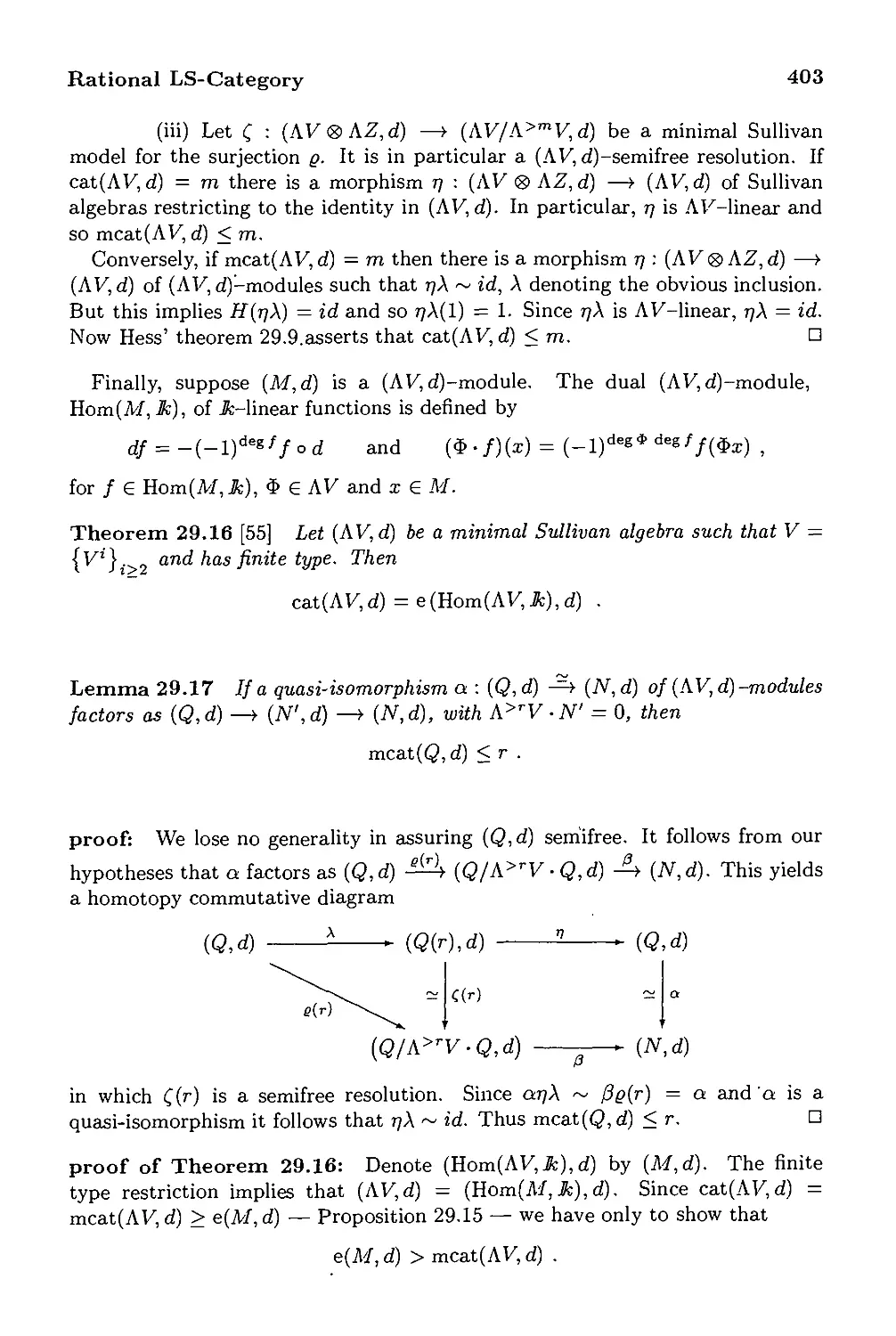
Part II: Sullivan Models
This Part is the core of the monograph, in which we identify the rational
homotopy theory of simply connected spaces with the homotopy theory of com-
mutative cochain algebras. This occurs in three steps:
• The construction in §10 of Sullivan's functor from topological spaces X to
commutative cochain algebras Api(X), which satisfies C*(X) ~ Api(X).
• The construction in §12 of the Sullivan model
(AV, d) A (A, d)
for any commutative cochain algebra satisfying H°(A,d) = k. (Here, fol-
lowing Sullivan ([144]), and the rest of the rational homotopy literature,
AV denotes the free commutative graded algebra on V.)
• The construction in §I7 of Sullivan's realization functor which converts
a Sullivan algebra, (AV, d), (simply connected and of finite type) into a
rational topological space |AV, d| such that (AV, d) is a Sullivan model for
APL(\AV,d\).
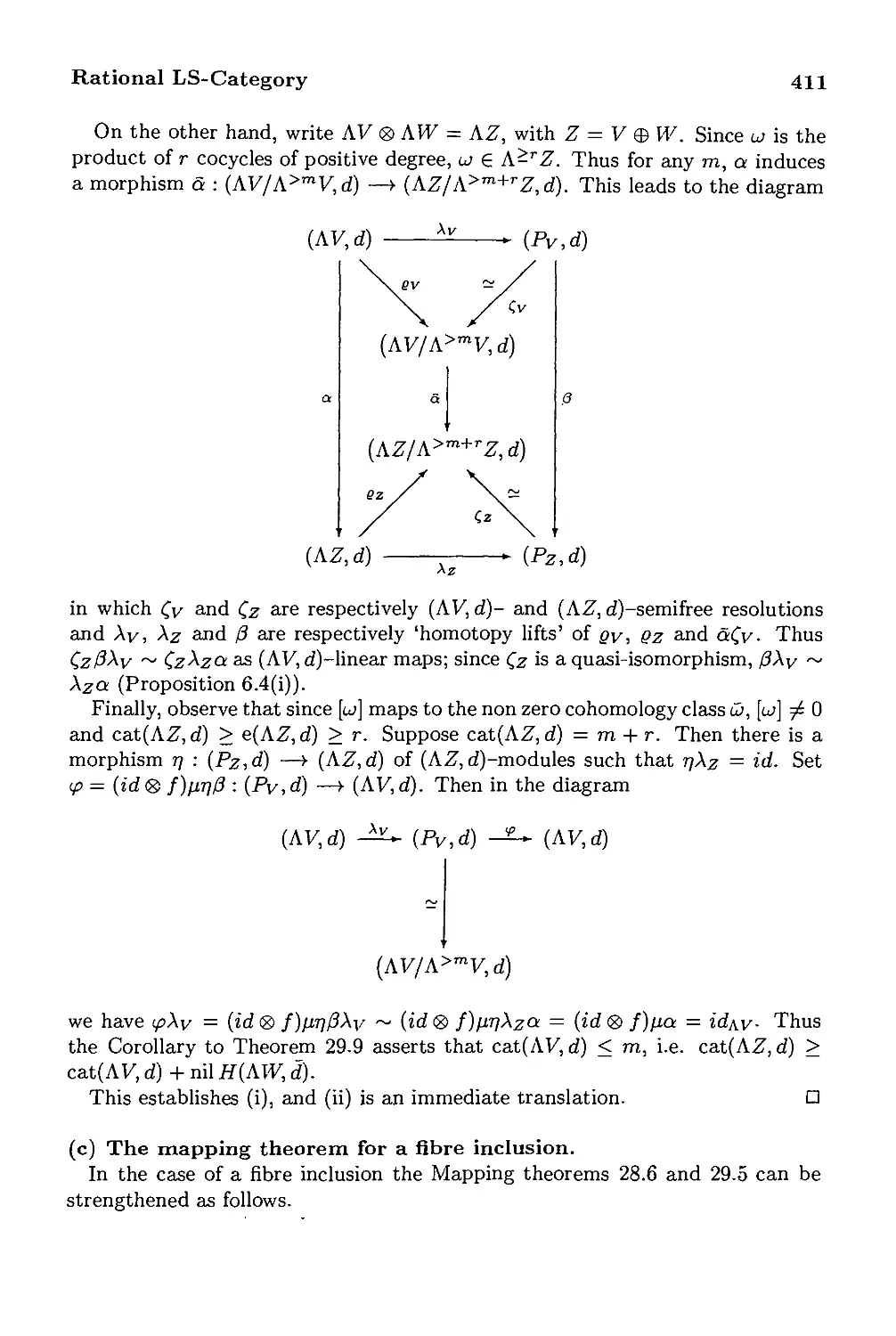
Along the way we show that these functors define bijections:
rational homotopy types =» f isomorphism classes of
of spaces J 1 minimal Sullivan algebras
and
, . , f s ( homotopy classes of
homotopy classes of I s I , . · ?
, . , > ¦<—*- < maps between minimal
maps between rational spaces I Inn· ? ?.
; ( Su livan algebras
Rational Homotopy Theory · x"i
where we restrict to spaces and cochain algebras that are simply connected with
cohomology of finite type.
Sullivan's functor Api was motivated by the classical commutative cochain
algebra Adr{M) of smooth differential forms on a manifold. In §11 we review
the construction of Adr(M) and prove Sullivan's result that Adr(M) is quasi-
isomorphic to Api(M;R). This implies (§12) that they have the same Sullivan
model.
The rest of Part II is devoted to the technology of Sullivan algebras, and to
geometric applications. We construct models of adjunction spaces, identity the
generating space V" of a Sullivan model with the dual of the rational homotopy
groups and identity the quadratic part of the differential with the dual of the
Whitehead product. Here the constructions are in §13 but some of the proofs
are deferred to §15.
In §14 we construct relative Sullivan algebras and decompose any Sullivan
algebra as the tensor product of a minimal and a contractible Sullivan algebra.
In §15 we use relative Sullivan algebras to model fibrations and show (applying
the result from §7) that the Sullivan fibre of the model is a Sullivan model for
the fibre. Finally, in §16 this material is applied to the structure of the homology
algebra H*{UX;]k) of the loop space of X.
Part III: Graded Differential Algebra (Continued).
In §3 we were careful to limit ourselves to those algebraic constructions needed
in Parts I and II. Now we need more: the bar construction of a cochain algebra,
spectral sequences (finally, we held off as long as possible!) and some elementary
homological algebra.
Part IV: Lie Models
In Part I we introduced the first of our algebraic categories (modules over
a dga), in Part II we focused on commutative cochain algebras and now we
introduce and study the third category, differential graded Lie algebras.
In §21 we introduce graded Lie algebras and their universal enveloping algebras
and exhibit the two fundamental examples in this monograph: the homotopy
Lie algebra L\ — ?»(??) (g> ifc of a simply connected topological space, and the
homotopy Lie algebra L of a minimal Sullivan algebra (i\V, d). The latter vector
space is defined by Lk = Horn [Vk+l, k) with Lie bracket given by the quadratic
part of d. Moreover, if (AV,d) is the Sullivan model for X then Lx s L.
In §22 we construct the free Lie models for a dgl, (L, d). We also construct (in
§22 and §23) the classical homotopy equivalences
(L,d)->C*(L,d) and {A, d) <~ ?{A4)
between the categories of dgl's (with L = L>! of finite type) and commutative
cochain algebras (with simply connected cohomology of finite type). In particular
a Lie model for a free topological space X is a free Lie model of ?(\v,d)i where
{AV, d) is a Sullivan model for X.
xiv Introduction
Given a dgl (L, d) that is free as a Lie algebra on generators ?; of degree n;
we show in §24 how to construct a CW complex X with a single (m + l)-cell
for each V{. and whose free Lie model is exactly (L.d). This provides a much
more geometric approach to the passage algebra —> topology then the realization
functor in §17.
Finally, §24 and §25 are devoted to Majewski's theorem [119] that if (L, d) is a
free Lie model for X then there is a chain algebra quasi-isomorphism U(L, d) -^4
C*(QX;Jk) which preserves the diagonals up to dga homotopy.
Part V: Rational Lusternik-Schnirelmann Category
The LS category, catX, of a topological space X is the smallest number m
(or infinity) such that X can be covered by m + 1 open sets each of which is
contractible in X. In particular:
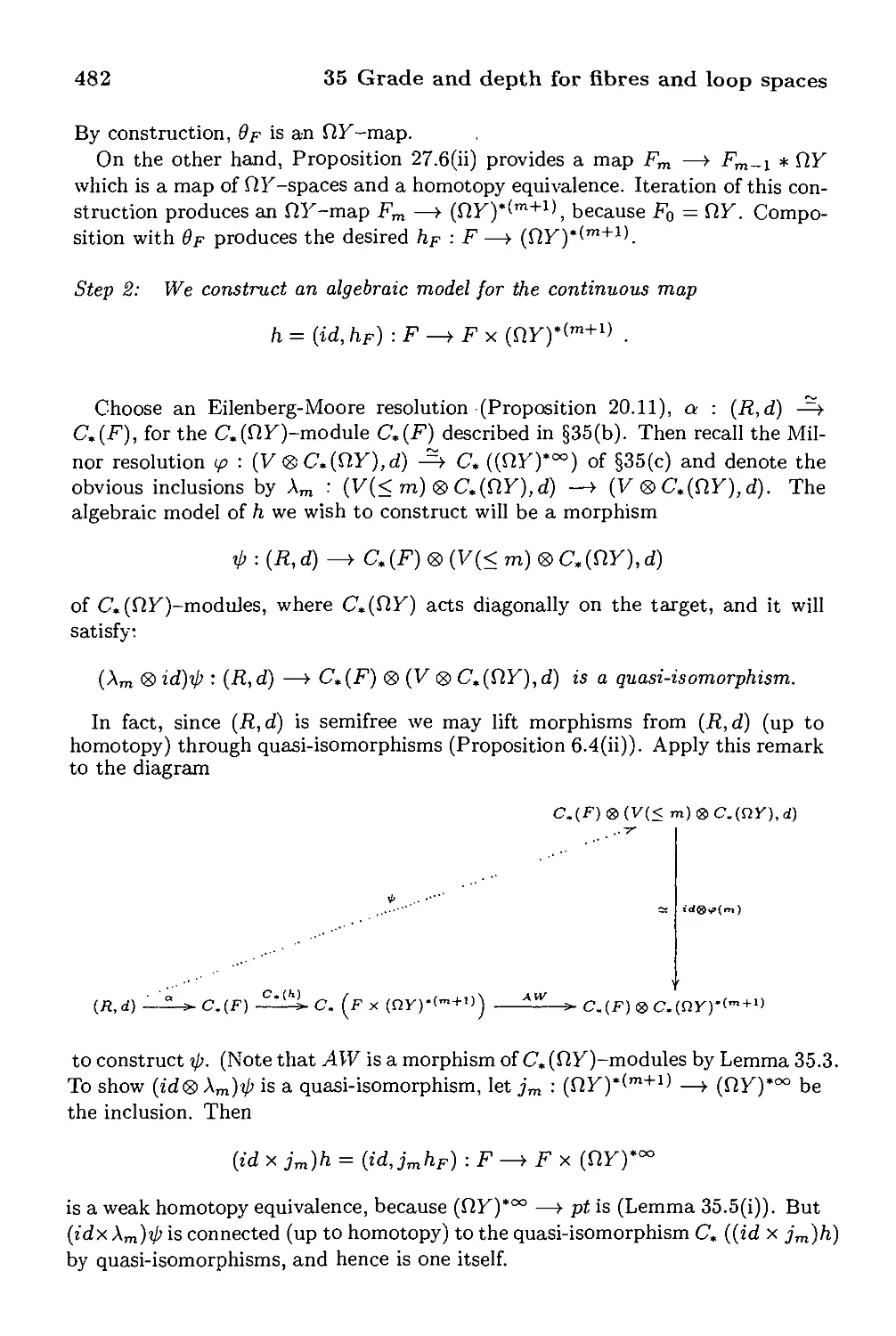
• caXX is an invariant of the homotopy type of X.
• // cat X = m then the product of any m + 1 cohomology classes of X is
zero.
• If X is a CW complex then catX < dimX but the inequality may be
oo
strict: indeed for the wedge of spheres X = V Sl we have dim X = oo and
cat* = 1.
The rational LS category, cato-X\ of X is the LS category of a rational CW
complex in the rational homotopy type of X.
Part V begins with the presentation in §27 of the main properties of LS cat-
egory for 'ordinary' topological spaces. We have included this material here for
the convenience of the reader and because, to our knowledge, much of it is not
available outside the original articles scattered through the research literature.
We then turn to rational LS category (§28) and its calculation in terms of
Sullivan models (§29). A key point is the Mapping Theorem: Given a continuous
map f : X —)¦ Y between simply connected spaces, then
?*(/) ® Q injective =? cat0 X < cato Y ¦
In particular, the Postnikov fibres in a Postnikov decomposition of a simply
connected finite CW complex all have finite rational LS category. (The integral
analogue is totally false!).
A second key result is Hess' theorem (Meat = cat), which is the main step in
the proof of the product formula cat^Q ? Yq = cat^Q + cati^ in §30. Finally,
in §31 we prove a beautiful theorem of Jessup which gives circumstances under
which the rational LS category of a fibre must be strictly less than that of the
total space of a fibration. The "?, ?" theorem described at the start of this
introduction is an immediate corollary.
Rational Homotopy Theory xv
Part VI: The Rational Dichotomy: Elliptic and Hyperbolic Spaces
AND Other Applications
In this Part we use rational homotopy theory to derive the results referred
to at the start of this introduction (and others) on the structure of ?*(???; k),
when X is a simply connected finite CW complex. These are outlined in the
introductions to the sections, and we leave it to the reader to check there, rather
than repeating them here.
As the overview above makes evident, this monograph makes no pretense of
being a complete account of rational homotopy theory, and indeed important
aspects have been omitted. For example we do not treat the iterated integrals
approach of Chen ([37], [79], [145]) and therefore have not been able to include
the deep applications to algebraic geometry of Hain and others (e.g. [80], [81],
[101]). Equivariant rational homotopy theory as developed by Triantafillou and
others ([151]) is another omission, as is any serious effort to treat the non-simply
connected case, even though at least nilpotent spaces are covered by Sullivan's
original theory. We have not described the Sullivan-Haefliger model ([144]. [78])
for the section space of a fibration even in the simpler case of mapping space,
except for the simple example of the free loop space Xs , nor have we included
the Sullivan-Barge classification ([144], [18]) of closed manifolds up to rational
homotopy type. And we have not given Lemaire's construction [108] of a finite
CW complex whose homotopy Lie algebra is not finitely generated as a Lie
algebra.
Moreover, this monograph does not pursue the connections outside or beyond
rational homotopy theory. Such connections include the algebraic homotopy the-
ory developed by Baues [20] following Quillen's homotopical algebra [134]. There
is no mention in the text (except in the problems at the end) of Anick's extension
of the theory to coefficients with only finitely many primes inverted ([11]) and its
application to loop space homology,and there is equally no mention of how the
results in Part VI generalize to arbitrary coefficients [56]. And finally, we have
not dealt with the interaction with the homological study of local commutative
rings [14] that has been so significantly exploited by Avramov and others.
We regret that limitations of time and energy (as well as our publisher's insis-
tence on limiting the number of pages!) have made it necessary simply to refer
the reader to the literature for these important aspects of the subject, in the
hope that what is presented here will make that task an easier one.
In the last twenty five years a number of monographs have appeared that
presented various parts of rational homotopy theory. These include Algebres
Connexes et Homologie des Espaces de Lacets by Lemaire [109], On PL de
Rham Theory and Rational Homotopy Type by Bousfield and Gugenheim ([30]),
Theorie Homotopique des Formes Differentielles by Lehmann ([107]), Rational
Homotopy Theory and Differential Forms by Griffiths and Morgan ([72]), Homo-
topie Rationnelle: Modeles de Chen, Quillen, Sullivan by Tanre ([145]), Lectures
on Minimal Models by Halperin [82], La Dichotomie Elliptique - Hyperbolique
en Homotopic Rationnelle by Felix ([50]), and Homotopy Theory and Models
xvi Introduction
by Aubry ([12]). Our hope is that the present work will complement the real
contribution these make to the subject.
This monograph brings together the work of many researchers, accomplished
as a co-operative effort for the most part over the last thirty years. A clear
account of this history is provided in [91], and we would like merely to indicate
here a few of the high points. First and foremost we want to stress our individual
and collective appreciation to Daniel Lehmann, who led the development of the
rational homotopy group at Lille that provided the milieu in which all three of us
became involved in the subject. Secondly, we want to emphasize the importance
of the memoir [109] by Lemaire and of the article [21] by Baues and Lemaire
which have formed the foundation for the use of Lie models.
In this context the mini-conference held at Louvain in 1979 played a key role.
It brought together the two approaches (Lie and Sullivan) and crystalized the
questions around LS category that proved essential in subsequent work. Another
mini-conference in Bonn in 1981 (organized jointly by Baues and the second
author) led to a trip to Sofia to meet Avramov and the intensification of the
infusion into rational homotopy of the intuition from local algebra begun by
Anick, Avramov, Lofwall and Roos.
This monograph was conceived of in 1992 and in the intervening eight years
we have benefited from the advice and suggestions of countless colleagues and
students. It is a particular pleasure to acknowledge the contributions of Cornea,
Dupont, Hess, Jessup, Lambrechts and Murillo, who have all worked with us as
students or postdocs and have all beaten problems we could not solve. We also
wish to thank Peter Bubenik whose careful reading uncovered an unbelievable
number of mistakes, both typographical and mathematical.
The actual writing and rewriting have been a team effort accomplished by the
three of us working together in intensive sessions at sites that rotated through
the campuses at which we have held faculty positions: the Universite Catholique
de Louvain (Felix), the University of Toronto and the University of Maryland
(Halperin) and the Universite de Lille 1 and the Universite d'Angers (Thomas).
The Fields Institute for Research in Mathematical Sciences in Toronto provided
us with a common home during the spring of 1996, and the Centre International
pour Mathematiques Pures et Appliquees, hosted and organized a two week sum-
mer school at Breil where we presented a first version of the text. Our granting
agencies (Centre National de Recherche Scientifique, FNRS, National Sciences
and Engineering Research Council of Canada, North Atlantic Treaty Organiza-
tion) all provided essential financial support. To all of these organizations we
express our appreciation.
Above all, however, we wish to express our deep gratitude and appreciation
to Lucile Lo, who converted thousands of pages of handwritten manuscript to
beautifully formatted final product with a speed, accuracy, intelligence and good
humor that are unparalleled in our collective experience.
Rational Homotopy Theory xvii
And finally, we wish to express our appreciation to our families who have lived
through this experience, and most especially to Agnes, Danielle and Janet for
their constant nurturing and support, and to whom we gratefully dedicate this
book.
Yves Felix
Steve Halperin
Jean-Claude Thomas
Contents
Introduction
Table of Examples
I Homotopy Theory, Resolutions for Fibrations, and ?-
?? cal Spaces
0 Topological spaces 1
1 CW complexes, homotopy groups and cofibrations 4
(a) CW complexes 4
(b) Homotopy groups 10
(c) Weak homotopy type 12
(d) Cofibrations and NDR pairs 15
(e) Adjunction spaces 18
(f) Cones, suspensions, joins and smashes 20
2 Fibrations and topological monoids 23
(a) Fibrations 24
(b) Topological monoids and G-fibrations 28
(c) The homotopy fibre and the holonomy action 30
(d) Fibre bundles and principal bundles 32
(e) Associated bundles, classifying spaces, the Borel construction and
the holonomy fibration 36
3 Graded (differential) algebra 40
(a) Graded modules and complexes 40
(b) Graded algebras 43
(c) Differential graded algebras 46
(d) Graded coalgebras 47
(e) When Jfe is a field 48
4 Singular chains, homology and Eilenberg-MacLane spaces 51
(a) Basic definitions, (normalized) singular chains 52
(b) Topological products, tensor products and the dgc, C*(X;Jfe). ... 53
(c) Pairs, excision, homotopy and the Hurewicz homomorphism .... 56
(d) Weak homotopy equivalences 58
(e) Cellular homology and the Hurewicz theorem 59
(f) Eilenberg-MacLane spaces 62
5 The cochain algebra Cm(X;k) 65
xx CONTENTS
6 (R, d)—modules and semifree resolutions 68
(a) Semifree models 68
(b) Quasi-isomorphism theorems 72
7 Semifree cochain models of a fibration 77
8 Semifree chain models of a G-fibration 88
(a) The chain algebra of a topological monoid 88
(b) Semifree chain models 89
(c) The quasi-isomorphism theorem 92
(d) The Whitehead-Serre theorem 94
9 P-local and rational spaces 102
(a) ¦P-local spaces 102
(b) Localization 107
(c) Rational homotopy type 110
II Sullivan Models
10 Commutative cochain algebras for spaces and simplicial sets 115
(a) Simplicial sets and simplicial cochain algebras 116
(b) The construction of A{K) 118
(c) The simplicial commutative cochain algebra -???, and Api(X) . . 121
(d) The simplicial cochain algebra Cpl, and the main theorem .... 124
(e) Integration and the de Rham theorem 128
11 Smooth Differential Forms 131
(a) Smooth manifolds 131
(b) Smooth differential forms 132
(c) Smooth singular simplices 133
(b) (d) The weak equivalence ADr(M) ~ APL(M;R) 134
12 Sullivan models 138
(a) Sullivan algebras and models: constructions and examples ..... 140
(b) Homotopy in Sullivan algebras 148
(c) Quasi-isomorphisms, Sullivan representatives, uniqueness of mini-
mal models and formal spaces 152
(d) Computational examples 156
(e) Differential forms and geometric examples 160
13 Adjunction spaces, homotopy groups and Whitehead products 165
(a) Morphisms and quasi-isomorphisms 166
(b) Adjunction spaces 168
(c) Homotopy groups 171
(d) Cell attachments 173
Rational Homotopy Theory xxi
(e) Whitehead product and the quadratic part of the differential ... 175
14 Relative Sullivan algebras 181
(a) The semifree property, existence of models and homotopy 182
(b) Minimal Sullivan models 186
15 Fibrations, homotopy groups and Lie group actions 195
(a) Models of fibrations 195
(b) Loops on spheres. Eilenberg-MacLane spaces and spherical fibrations200
(c) Pullbacks and maps of fibrations 203
(d) Homotopy groups 208
(e) The long exact homotopy sequence 213
(f) Principal bundles, homogeneous spaces and Lie group actions . . . 216
16 The loop space homology algebra 223
(a) The loop space homology algebra 224
(b) The minimal Sullivan model of the path space fibration 226
(c) The rational product decomposition of UX 228
(d) The primitive subspace of H*{UX\k) 230
(e) Whitehead products, commutators and the algebra structure of
H*{9.X;k) 232
17 Spatial realization 237
(a) The Milnor realization of a simplicial set 238
(b) Products and fibre bundles 243
(c) The Sullivan realization of a commutative cochain algebra 247
(d) The spatial realization of a Sullivan algebra 249
(e) Morphisms and continuous maps 255
(f) Integration, chain complexes and products 256
III Graded Differential Algebra (continued)
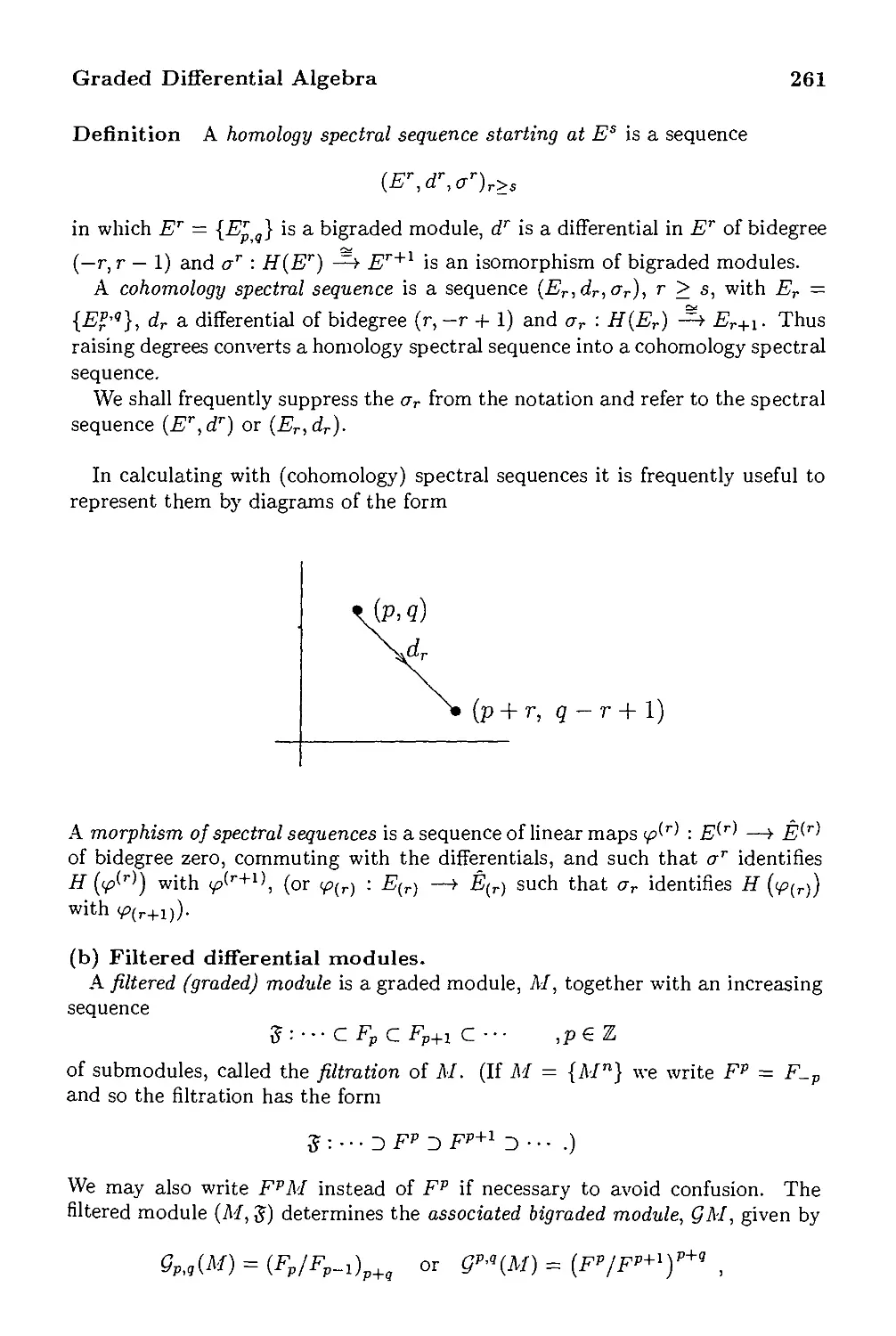
18 Spectral sequences 260
(a) Bigraded modules and spectral sequences 260
(b) Filtered differential modules 261
(c) Convergence 263
(d) Tensor products and extra structure 265
19 The bar and cobar constructions 268
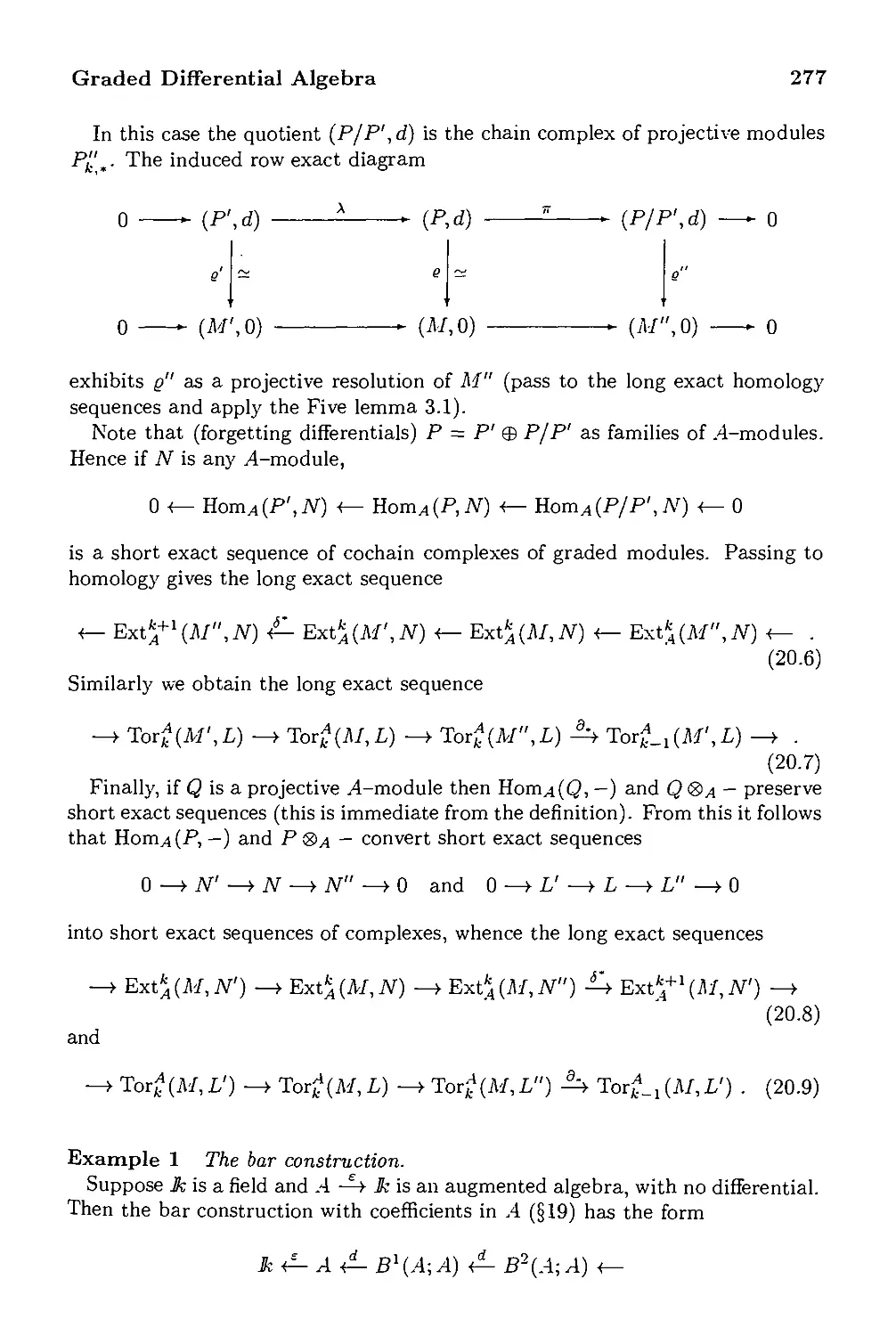
20 Projective resolutions of graded modules 273
(a) Projective resolutions 273
(b) Graded Ext and Tor 275
(c) Projective dimension .'! 278
(d) Semifree resolutions 278
xxii CONTENTS
IV Lie Models
21 Graded (differential) Lie algebras and Hopf algebras 283
(a) Universal enveloping algebras 285
(b) Graded Hopf algebras 288
(c) Free graded Lie algebras 289
(d) The homotopy Lie algebra of a topological space 292
(e) The homotopy Lie algebra of a minimal Sullivan algebra 294
(f) Differential graded Lie algebras and differential graded Hopf algebras296
22 The Quillen functors C. and C 299
(a) Graded coalgebras 299
(b) The construction of C»(L) and of C*(L;M) 301
(c) The properties of C.(L;UL) 302
(d) The quasi-isomorphism C*{L) ~ ) BUL 305
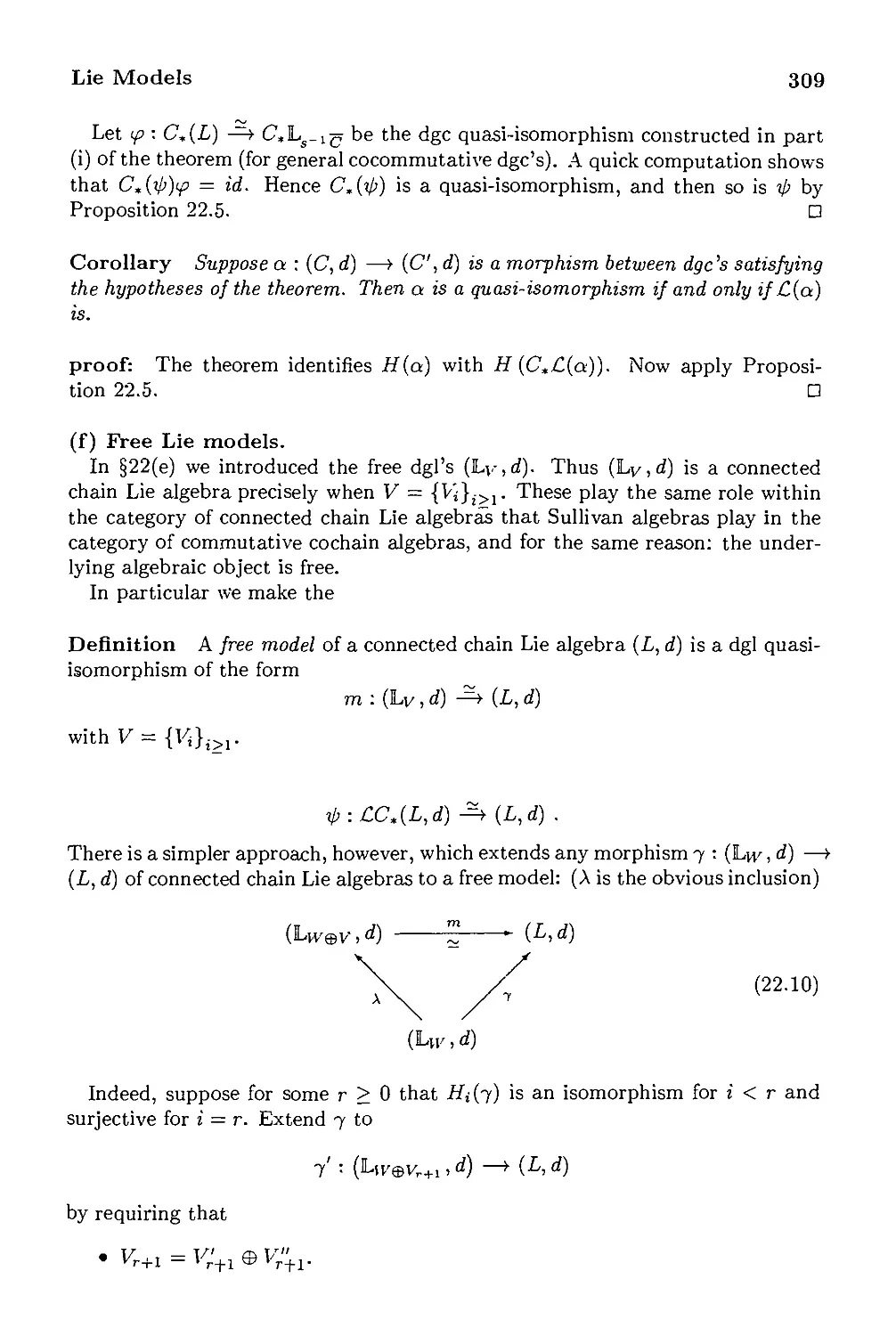
(e) The construction C{C,d) 306
(f) Free Lie models , . 309
23 The commutative cochain algebra, C*{L,dL) 313
(a) The constructions C*(L,di), and ?(/t,<f) 313
(b) The homotopy Lie algebra and the Milnor-Moore spectral sequence 317
(c) Cohomology with coefficients 319
24 Lie models for topological spaces and CW complexes 322
(a) Free Lie models of topological spaces 324
(b) Homotopy and homology in a Lie model 325
c) Suspensions and wedges of spheres 326
(d) Lie models for adjunction spaces 328
(e) CW complexes and chain Lie algebras 331
(f) Examples 331
(g) Lie model for a homotopy fibre 334
25 Chain Lie algebras and topological groups 337
(a) The topological group, \TL\ 337
(b) The principal fibre bundle, 338
(c) \TL\ as a model for the topological monoid, QX 340
(d) Morphisms of chain Lie algebras and the holonomy action 341
26 The dg Hopf algebra C*{QX) 343
(a) Dga homotopy 344
(b) The dg Hopf algebra C» (UX) and the statement of the theorem . 346
(c) The chain algebra quasi-isomorphism ? : (ULv,d) 347
id) The proof of Theorem 26.5 349
Rational Homotopy Theory xxiii
V Rational Lusternik Schnirelmann Category
27 Lusternik-Schnirelmann category 351
(a) LS category of spaces and maps 352
(b) Ganea's fibre-cofibre construction 355
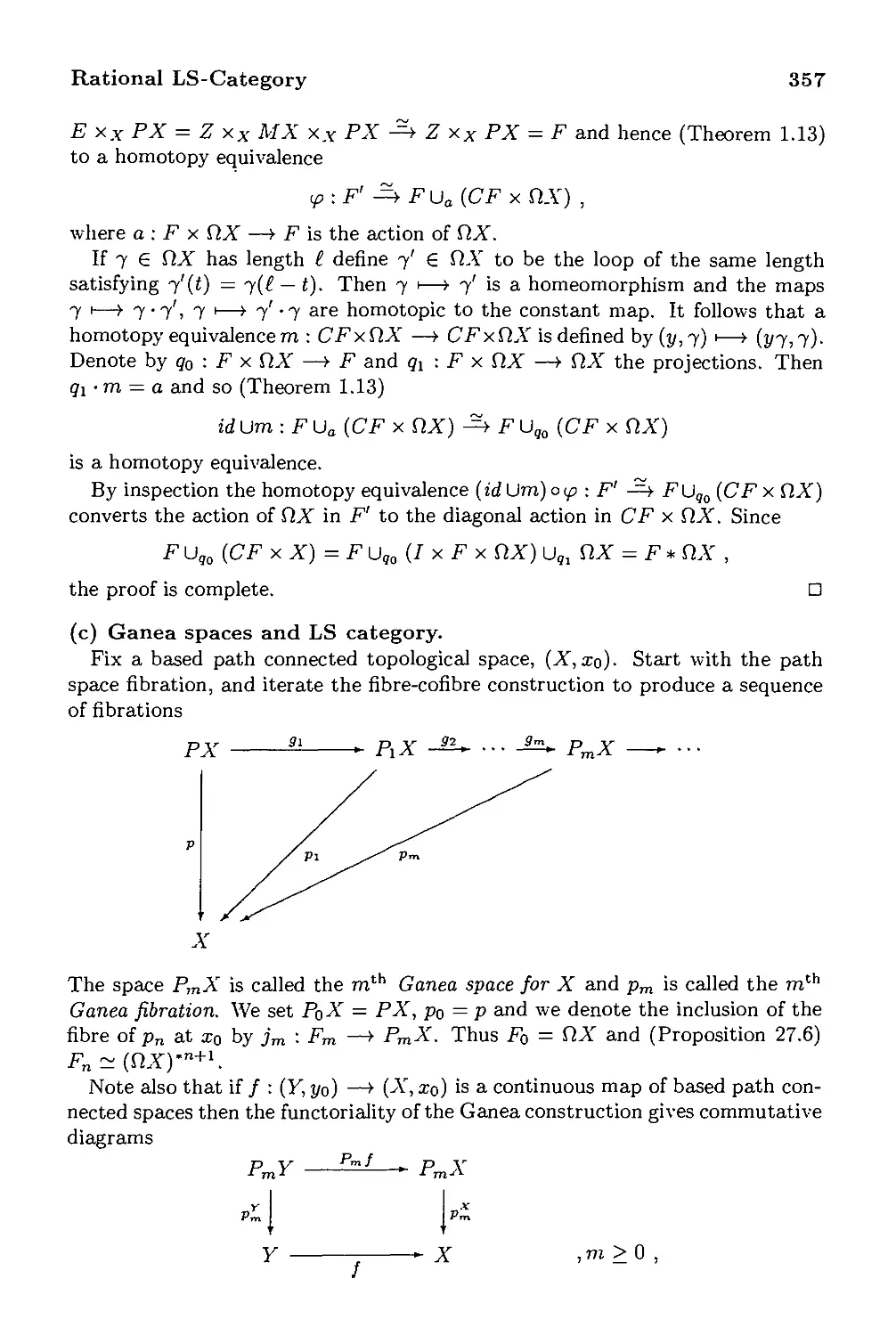
(c) Ganea spaces and LS category 357
(d) Cone-length and LS category: Ganea's theorem 359
(e) Cone-length and LS category: Cornea's theorem 361
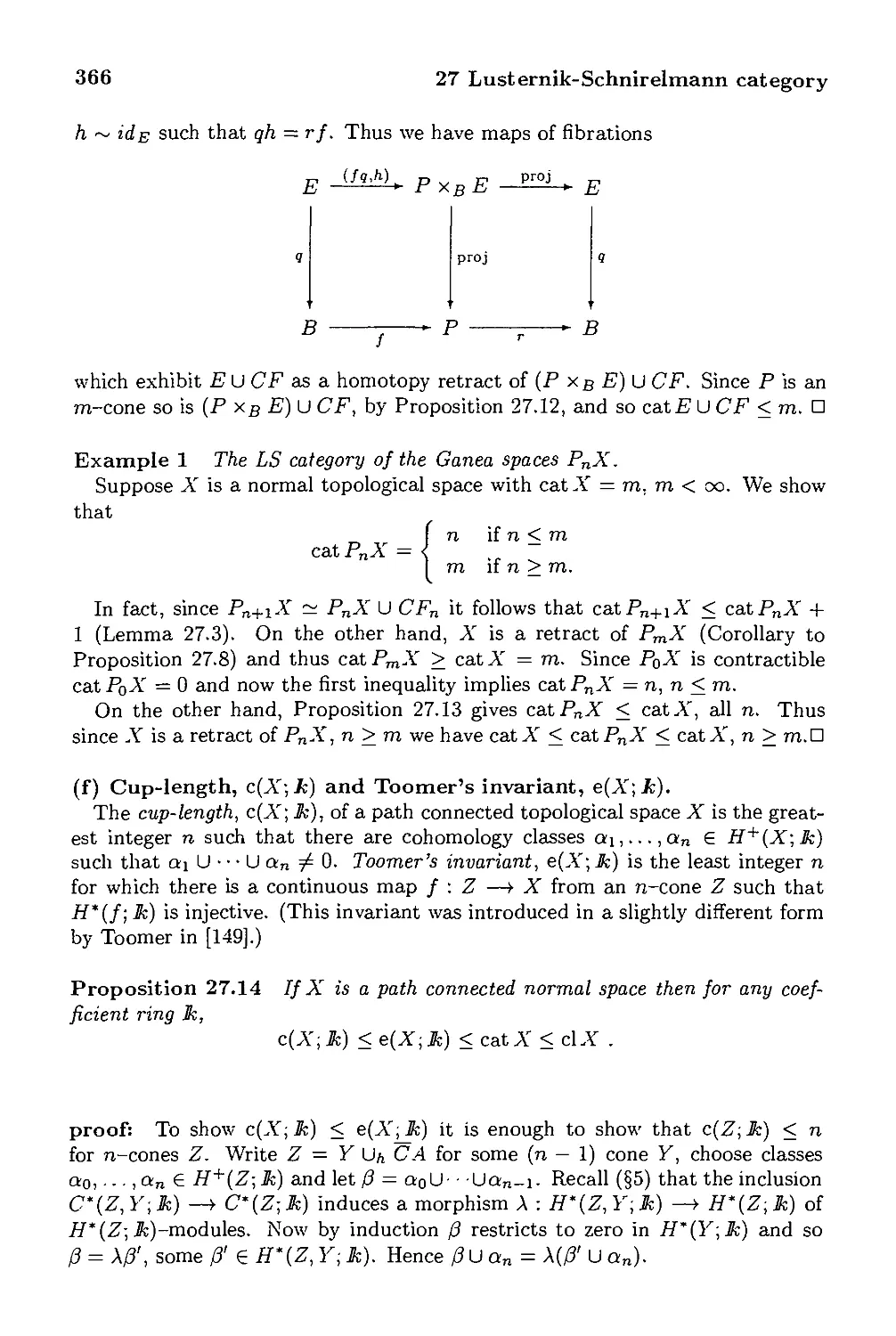
(f) Cup-length, c(X;Jk) and Toomer's invariant, e(A';Jc) 366
28 Rational LS category and rational cone-length 370
(a) Rational LS category 371
(b) Rational cone-length 372
(c) The mapping theorem 375
(d) Gottlieb groups 377
29 LS category of Sullivan algebras 381
(a) The rational cone-length of spaces and the product length of models 382
(b) The LS category of a Sullivan algebra 384
(c) The mapping theorem for Sullivan algebras 389
(d) Gottlieb elements 392
(e) Hess' theorem 393
(f) The model of (AV,d) -> {AV/A>mV,d) 396
(g) The Milnor-Moore spectral sequence and Ginsburg's theorem . . . 399
(h) The invariants meat and e for (AV, d)-modules 401
30 Rational LS category of products and fibrations 406
(a) Rational LS category of products 406
(b) Rational LS category of fibrations 408
(c) The mapping theorem for a fibre inclusion 411
31 The homotopy Lie algebra and the holonomy representation 415
(a) The holonomy representation for a Sullivan model 418
(b) Local nilpotence and local conilpotence 420
(c) Jessup's theorem 424
(d) Proof of Jessup's theorem 425
(e) Examples 430
(f) Iterated Lie brackets 432
VI The Rational Dichotomy:
Elliptic and Hyperbolic Spaces
and
Other Applications
32 Elliptic spaces 434
xxiv CONTENTS
(a) Pure Sullivan algebras 435
(b) Characterization of elliptic Sullivan algebras 438
(c) Exponents and formal dimension 441
(d) Euler-Poincare characteristic 444
(e) Rationally elliptic topological spaces 447
(f) Decomposability of the loop spaces of rationally elliptic spaces . . 449
33 Growth of Rational Homotopy Groups 452
(a) Exponential growth of rational homotopy groups 453
(b) Spaces whose rational homology is finite dimensional 455
(c) Loop space homology 458
34 The Hochschild-Serre spectral sequence 464
(a) Horn, Ext, tensor and Tor for i7L-modules 465
(b) The Hochschild-Serre spectral sequence 467
(c) Coefficients in UL 469
35 Grade and depth for fibres and loop spaces 474
(a) Complexes of finite length '. 475
(b) nr-spaces and C*(n7)-modules 476
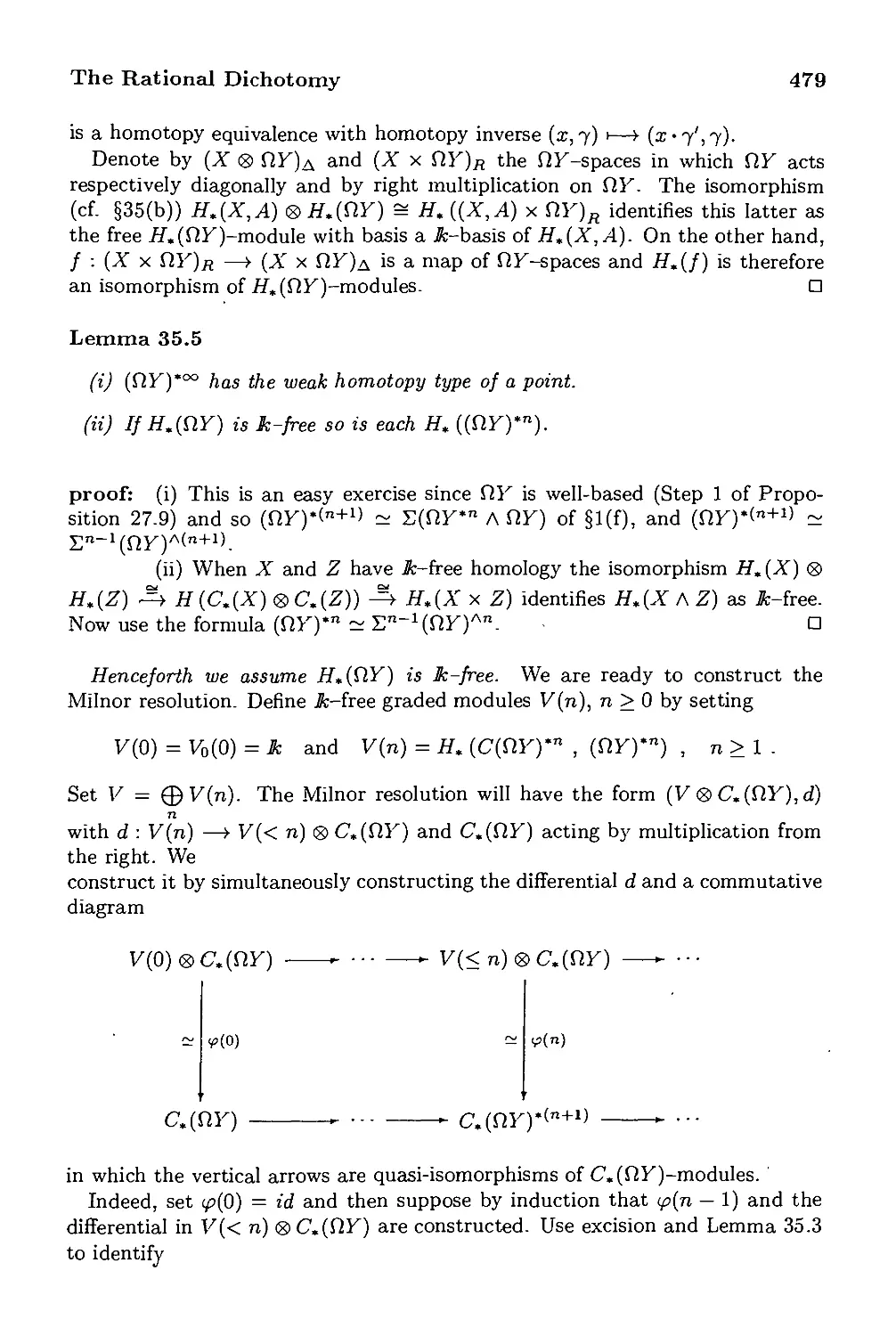
(c) The Milnor resolution oik 478
(d) The grade theorem for a homotopy fibre 481
(e) The depth of if, (??) 486
(f) The depth of UL 486
(g) The depth theorem for Sullivan algebras 487
36 Lie algebras of finite depth 492
(a) Depth and grade 493
(b) Solvable Lie algebras and the radical 495
(c) Noetherian enveloping algebras 496
(d) Locally nilpotent elements 497
(e) Examples 497
37 Cell Attachments 501
(a) The homology of the homotopy fibre, X xy PY 502
(b) Whitehead products and G-fibrations 502
(c) Inert element 503
(d) The homotopy Lie algebra of a spherical 2-cone 505
(e) Presentations of graded Lie algebras 507
(f) The Lofwall-Roos example 509
38 Poincare Duality 511
(b) Properties of Poincare duality 511
(b) Elliptic spaces 512
(c) LS category 513
(c\) Inert elements 513
Rational Homotopy Theory *xv
39 Seventeen Open Problems 516
References 521
Index 531
Table of Examples
§1. CW complexes, homotopy groups and cofibrations
(a) 1. Complex projective space
2. Wedges
3. Products
4. Quotients and suspensions
?. Cubes and spheres
6. Adjunction spaces
7. Mapping cylinders
§2. Fibrations and topological monoids
(a) 1. Products
2. Covering projections
3. Fibre products and pullbacks
(b) 1. Path space fibrations
§3. Graded (differential) algebra
(b) 1. Change of algebra
2. Free modules
3. Tensor product of graded algebras
4. Tensor algebra
5. Commutative graded algebras
6. Free commutative graded algebras
(c) 1. Horn* (M,N)
2. Tensor products
3. Direct products
4. Fibre products
§9. "P-local and rational spaces
(a) 1. The infinite telescope S™
(b) 1. Cellular localization
§10. Commutative cochain algebras for spaces and simplicial set
(c) 1. APL(pt)
§11. Smooth differential forms
(a) Smooth manifolds:
1. W1
2. Open subsets
3. Products
xxviii Table of Examples
§12. Sullivan models
(a) 1. The spheres, Sk
2. Products of topological spaces
3. //-spaces
4. A cochain algebra (AV, d) that is not a Sullivan algebra
5. (AV,d),lmdcA+V-A+V
6. Topological spaces with finite homology admit finite dimensional
commutative models
7. (A(a, b, x. y, z),d), da = db = 0, dx = a2, dy = ab, dz = b2
(b) 1. Null homotopic morphisms into (^4,0) are constant
(c) 1. Wedges
(d) 1. (AV, d) = A(x,y,z;dx = dy = 0, dz = xy), deg ? = 3, deg y = 5
2. Automorphisms of the model of S3 ? S3 ? Ss ? S6
3. (AV,d) = ?(?, ?, b;da = dx = 0,db = ax), dega = 2, dega: = 3
4. A(x,y,z,u;dx = dy = dz = 0,du = xm + ym - zm), deg a: =
degy = degz = 2
5. Two morphisms that homotopy commute, but are not homotopic
to commuting morphisms
6. Sullivan algebras homogeneous with respect to wordlength
7. Sullivan models for cochain algebras (H, 0) with trivial multipli-
cation
(e) 1. The cochain algebra Aor(G)g of right invariant forms on G, and
its minimal model
2. Nilmanifolds
3. Symmetric spaces are formal
4. Compact Kahler manifolds are formal
5. Symplectic manifolds need not be formal
§13. Adjunction spaces, homotopy groups and Whitehead products
(e) 1. The even spheres S2n
2. S3 V S3 U/ (?>g II ?>?)
§14. Relative Sullivan algebras
(b) 1. The acyclic closure (B <8> AV, d)
2. A commutative model for a Sullivan fibre
§15. Fibrations, homotopy groups and Lie group actions
(b) 1. The model of the loop space ClSk, k>2
2. The model of an Eilenberg-MacLane space
3. The rational homotopy type of fc(Z,n)
? Qr^Vipriral fibrations
Rational Homotopy Theory xxix
5. Complex projective spaces
(c) 1. The free loop space Xs
(d) 1. Rational homotopy group of spheres
2. (A(eo,ei,x),dx = eoei)
3. The Quilleri plus construction
4. Smooth manifolds and smooth maps
(f) 1. Homogeneous spaces
2. Borel construction; application to free actions
3. Matrix Lie groups
4. A fibre bundle with fibre S3 x SUC) that is not principal
5. Non-existence of free actions
§16. The loop space homology algebra
(a) 1. The homology algebra if. (US2k+1 ; Jfe), k > 1
(e) 1. The homology algebra if, (USn+i ; Jfe)
§17. Spatial realization
(a) 1. |?[?]| = ?"
2. Cones
3. Extendable simplicial sets
(b) 1. A simplicial construction of the Eilenberg MacLane space K(k, 1)
(c) 1. Realization of products
2. Contractible Sullivan algebras have contractible realizations
§18. Spectral sequences
(b) 1. Semifree modules
2. Relative Sullivan algebras
3. The wordlength filtration of a Sullivan algebra
4. Bigradations
(d) 1. Filtered differential graded algebras
§20. Projective resolutions of graded modules
(b) 1. The bar construction
(d) 1. The Eilenberg-Moore spectral sequence
§21. Graded (differential) Lie algebras and Hopf algebras
1. Graded algebras
2. The adjoint representation
3. Derivations
4. Products
5. The tensor product .4 <8> L
xxx Table of Examples
(a) 1. Abelian Lie algebras
§22. The commutative cochain algebra C*(L,cIl)
(a) 1. Graded Lie algebras
2. Free graded Lie algebras
3. Minimal Lie models of minimal Sullivan algebras
4. Sullivan algebras (AW,d) for which HQk(AW,d) = 0, 1 < k < ?
(b) 1. C*(L,dL)
2. Topological spaces X
§24. Lie models for topological spaces and CW complexes.
1. The free Lie model of a sphere
(c) 1. If H+(X; Q) is concentrated in odd degrees then D has the ratio-
nal homotopy type of a wedge of spheres.
(f) 1. (Ly,0) is the minimal Lie model of a wedge of spheres
2. The coproduct of Lie models is a Lie model for a wedge
3. The direct sum of Lie models is a Lie model of the product
4. A Lie model for S3 ? So3 \J[*,[O,0]W)W D8
5. Lie models for CP°° and CP"
6. A Lie model for (CP2 V S3) \J[o>0]w Ds
7. Coformal spaces
8. Minimal free chain Lie model
§27. Lusternik-Schnirelmann category
(e) 1. The LS category of the Ganea spaces PnX
§28. Rational LS category and rational cone-length
(a) 1. cato I V^a ) = max{cato(Xa))
(c) 1. Postnikov fibres
2. Free loop spaces have infinite rational category
(d) 1. G-spaces
2. The holonomy fibration
3. Loop spaces
§29. LS category of Sullivan algebras
(b) 1. A space X satisfying c0X < e0X
2. A space X satisfying e^X < cat0 X
3. A space X satisfying cat0 X < do X
4. Formal spaces
5 Coformal spaces
'•?, ? „At]n v _ yodd anddim
Rational Homotopy Theory xxxi
(c) 1. e ((AV, d) ® (kW, d)) = e(AV, d) + e{AW, d)
2. cat0 X - e0X can be arbitrary large
(d) 1. A non-trivial Gottlieb element
(e) 1. Field extension preserves category
2. Rational category of smooth manifolds
§30. Rational LS category of products and fibrations
(b) 1. The relative Sullivan algebra (i\.(x,y),dy = xr+1)
—> (? (?, y, u, v),dy = xr+1, dv = un+1 — x)
(c) 1. Fibrations with cat X = 0
2. Fibrations with cat X = 1
3. Fibrations with cat0 X = max(cat0 Y, cat0 F)
§31. The homotopy Lie algebra and the holonomy representation
(b) 1. The holonomy representation
2. The adjoint representation
(e) 1. The fibration ? : S4m+3 —> MPm
2. The fibration associated with S3 V S3 -^ S3
3. Derivations in commutative cochain algebras
4. A fibration X —> CPn with fibre S3 and cat0 X = 2
(f) 1. A space X with eoX = 2 and cat0X = oo
§32. Elliptic spaces
(b) 1. A(a2,X3,u3,b4,v5,w7] da = dx = 0, du = ?2, db = ??, di» =
?& — ux, dw = b2 — vx)
2. Adding variables of high degree
3. Algebraic closure of Jfc is necessary in Proposition 32.3
4. ?-colourable graphs
(e) 1. Simply connected finite ??-spaces are rationally elliptic
2. Simply connected homogeneous spaces G/K are rationally elliptic
3. Free torus actions
§33. Growth of rational homotopy groups
(c) 1. Wedges of spheres
2. X = S3VS3
3. X = S3VS32U[a[a>?]w]wDs
4. X = T{S3,S3,S3)
5. X = YVZ
§34. The Hochschild-Serre spectral sequence
(a) 1. Torul(lk,]k) = s (//[/,/])
2. The representation of L/I in Tor^Jc, Jfc)
xxxii Table of Ex
§36. Lie algebra of finite depth
(e) 1. Depth = 0
2. Free products have depth 1
3. ivy
4. Products
5. X=S*aVS!l)[a[a>b]w]wD&
6- CP°°/CPn
7. eo(X) = 2; cato(JQ = depth Lx = 3
8. L = Der>0 Ly, where F is a finite dimensional vector
dimerision at least 3
Part I
Homotopy Theory, Resolutions
for Fibrations, and P-local Spaces
0 Topological spaces
In this section we establish the notation and conventions for topological spaces
that will obtain throughout the monograph.
A k-space (not to be confused with the symbol k used to denote coefficient
ring) is a Hausdorff topological space ?' such that Aclis closed if and only
if ? ? C is closed in C for all compact subspaces C C X. If X is any Hausdorff
topological space then Xk is the set X equipped with the associated k-space
topology: A is closed in Xk if and only if ? ? C is closed in C for all compact
subspaces C oi X. It is easy to see that Xk is a fc-space, Xk -^ X is a continuous
bijection and X = Xk if and only if X itself is a fc-space. Moreover, all metric
spaces are fc-spaces. A continuous map / : X —» Y is proper if f~1(C) is
compact whenever C is. It is an easy but important observation that: ? proper
continuous bijection between k-spaces is a homeomorphism.
Henceforth in this book we shall restrict attention to k-spaces.
Consistent with this convention we need to modify some (but not all) of the
standard topological constructions as follows [44].
• Subspaces.
If .A is a subset of a fc-space X then we assign to A the &-space topology
associated with the 'ordinary' subspace topology.
• Products.
If {Xa} is a family of fc-spaces we assign to the set theoretic product Y[Xa
a
the fc-space topology associated to the ordinary product topology. If ? is any
fc-space then a map / : ? —» f] Xa is continuous if and only if each 'component'
a
fa : ? —> Xa is continuous.
Note also that if X is locally compact and Y is a fc-space then the fc-space
topology in ? ? ? is just the ordinary topology.
• Quotients.
A quotient space F of I is a surjection ? : X —t Y such that U C Y is
open if and only if p~l(U) is open. We only consider quotients Y such that Y
is Hausdorff and X is a fc-space; in this case Y is automatically a fc-space. The
product pxp' : X xX1 —» ? ? ?' of two such quotient maps is itself a quotient
map. (This follows easily from a lemma of Whitehead [44] which states that
? ? id : ? ? ? —> ? ? ? is a quotient map if ? is locally compact.)
• Mapping spaces.
If Ar and Y are topological spaces then Yx (as a set) is the set of continuous
maps from X to Y. The compact-open topology in this set is the topology whose
open sets are the arbitrary unions of finite intersections of subsets of the form
U with U open in Y and C compact in X. As usual we assign to Yx the k-
space topology associated with the compact-open topology. Then for fe-spaces
2 0 Topological spaces
?, ?, ? the exponential law asserts that a homeomorphism ZYxX -=» (Zy )x
is given by / *-? F, with F (?) (y) — /(y, ?). In particular, the evaluation map
Yx ? X —>¦ ?, (g,x) *-? g(x), is continuous.
The following conventions from topology will be used without further refer-
ence.
• The length of ? G Rk is denoted by ||u||.
• The unit interval I — [0,1].
• The unit ?-cube In = / ? · · · ? / C ?". Thus the boundary, dln, consists
of the points ? G /" with some x,· G {0,1}.
• The ?-dimensional disk Dn C W1 is defined by Dn = {x G W1 \\x\\ < 1}.
• The ?-dimensional sphere Sn C Rn+1 is defined by 5" = {x G E"+1
||z|| = 1}. Thus 5" = dDn+1 is the boundary of the (n + l)-disk.
• A pair of topological spaces, (X, A) is a space X and a subspace A C X
(with the &-space topology described above).
A map of pairs, ? : {?,?) -> (?, ?) is a continuous map ? ? : ? ->¦ Y
restricting to ? a : A ->· ?. If ? is another space then
(?',?) ?? = (? ??,? ??).
• Given continuous maps X ·—> Y ^- ? the fibre product X xy ? C X x ?
is the subspace of points (x, z) such that f(x) = 5B). Projection defines a
commutative square
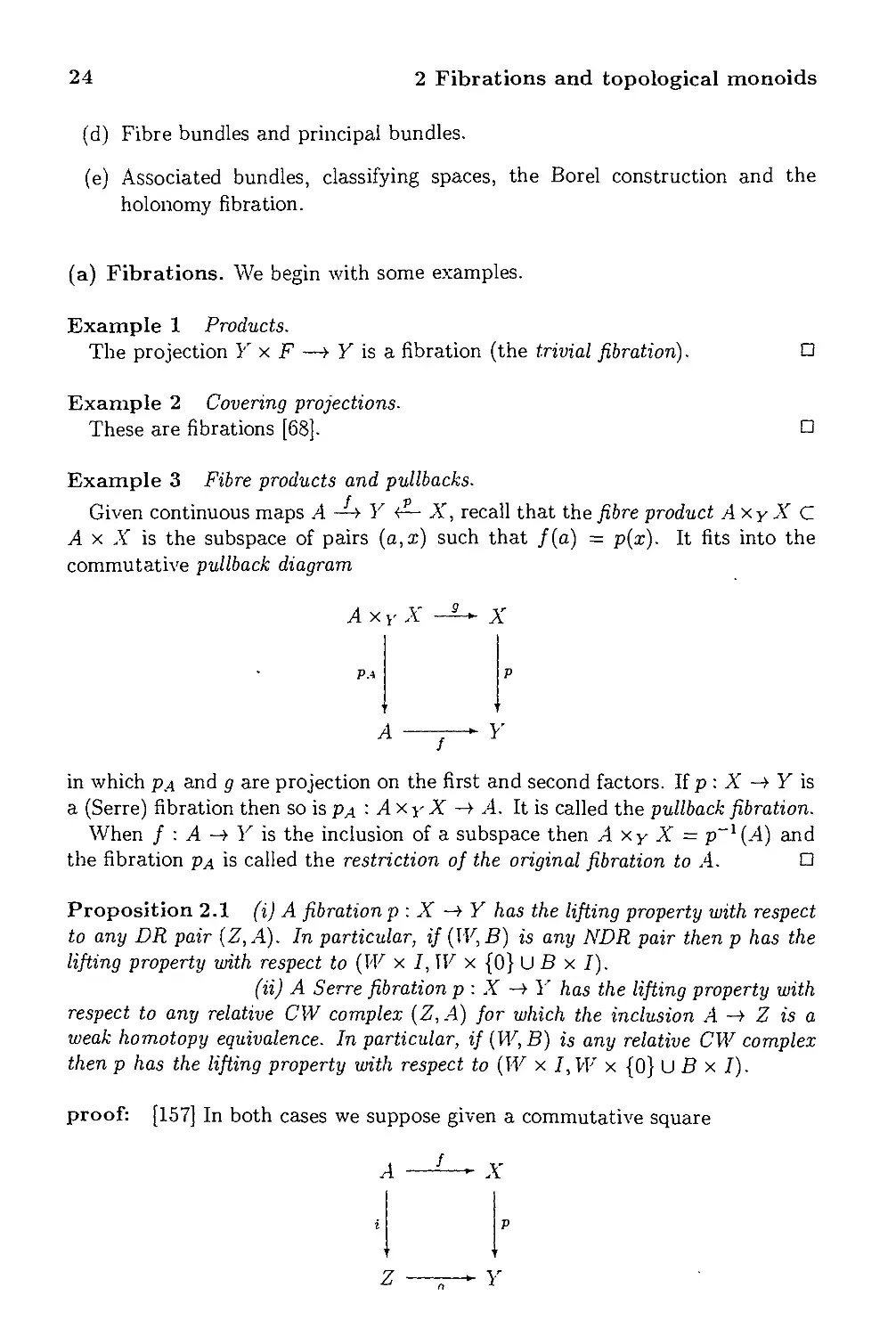
X xy ? —- ?
?—r-?
and any pair of continuous maps ? : W ¦—> ?, -? : W —>¦ ? such that
?? — gu defines a continuous map (?, ?) :W —>¦ ? ?? ?.
A based (or pointed) space is a pair (X,x0); x0 e X is the basepoint. A
based map is a map </? : (?,??) -> (y, yo).
The disjoint union of spaces Xa is denoted by ]J Xa. The we^e of based
Q
spaces (Xa,xa) is the based space \/QXa = Uxa/U.{x<*}- Based maps
"i : (X.xn) —> {Z,z0) and t/>(y, j/0) —* (Z,z0) define a map (?, ?) : A" V
Homotopy Theory 3
• Suppose given a pair (Z,B) and a continuous map f : ? -> X. We
denote by X U/ ? the quotient space of A" JJ ? obtained by identifying
b ~ f(b),b G ?. It is called the adjunction space obtained by attaching ?
to X along /.
• Given A C X the based space {X/A, [A]) is obtained by identifying the
points of .4 to a single point [A], and giving X/A the quotient topology.
Thus X/A = * U/ X, where f : A -l· * is the constant map.
• The suspension, ??, of a based space (A', xo) is the based space A" x
7/(A"x{0,l}U{zo} ?/)·
• Continuous maps f,g : X -> Y are homotopic (/ ~ 5) if there is a continu-
ous map F : ? ? I ^ Y such that F(x, 0) = /(x) and F(x, 1) = c/(x), ? G
A. F is a homoEopy from / to g. Being homotopic is an equivalence
relation; the equivalence class of / is its homotopy class and the set of
homotopy classes of maps is denoted by [X, Y].
• A homotopy equivalence is a continuous map / : X ¦—> Y such that for
some continuous map g : Y ¦—> I we have fg ~ id ? and gf ~ id ?. In
this case we write / : A -=> Y and we say g is a homotopy inverse for /.
If there is a homotopy equivalence from A to y then A' and Y have the
some homotopy type and we write A ~ y. If the constant map A ·—> pi
is a homotopy equivalence then X is contractible.
• A tosec? homotopy between /, g : (A, x0) —> (Y,yo) is a continuous map
F : (?,??) ? ? -> (Y,y0) such that F(x,0) = /(x) and F(x,l) = g(x)·
This is an equivalence relation and the class of / is its based homotopy
class. The set of based homotopy classes is denoted by [(A,xo), (Y, j/o)]·
A tosec? homotopy equivalence is a continuous map / : (A, x0) ·—> (Y, J/o)
such that for some continuous map g : (Y, j/o) ¦—> (?',??), fg and 5/ are ,
respectively, based homotopic to idy and id ?.
• Two continuous maps /, g : X -> Y which restrict to the same map ? in a
subspace A C A are homotopic rel .4 if there is a homotopy ? from / to
g such that H(a,t) = </?(a) for all ? G .4, t G /; H is a homotopy rel .4.
• A subspace .<4C—>A is a retract of A if there is a map ? : A" ->· .4 (the
retraction) such that pi = id.4. It is a strong deformation retract if also
ip ~ idx rel A.
1 CW complexes, homotopy groups and cofibra-
tions
We begin this monograph by introducing the main objects of study in homo-
topy theory: CW complexes and their homotopy groups. The topological spaces
(manifolds, polyhedra, real algebraic varieties, ... ) that arise in geometric con-
texts are all CW complexes.
Homotopy groups are groups ??(?), ? > 1, defined for any topological space,
and abelian for ? > 2. A weak homotopy equivalence is a continuous map / such
that 7?*(/) is an isomorphism, and we establish two important results of JHC
Whitehead:
• There is a weak homotopy equivalence from a CW complex to any topolog-
ical space, and
• A continuous map from a CW complex lifts, up to homotopy, through a
weak homotopy .equivalence.
It follows that two CW complexes connected by a chain of weak homotopy equiv-
alences have the same homotopy type.
This section is organized into the following topics (We note however that, aside
from the definition of suspension, topics (d) - (f) will not be needed until Part
V):
(a) CW complexes.
(b) Homotopy groups.
(c) Weak homotopy type.
(d) Cofibrations and NDR pairs.
(e) Adjunction spaces.
(f) Cones, suspensions, joins and smashes.
(a) CW complexes.
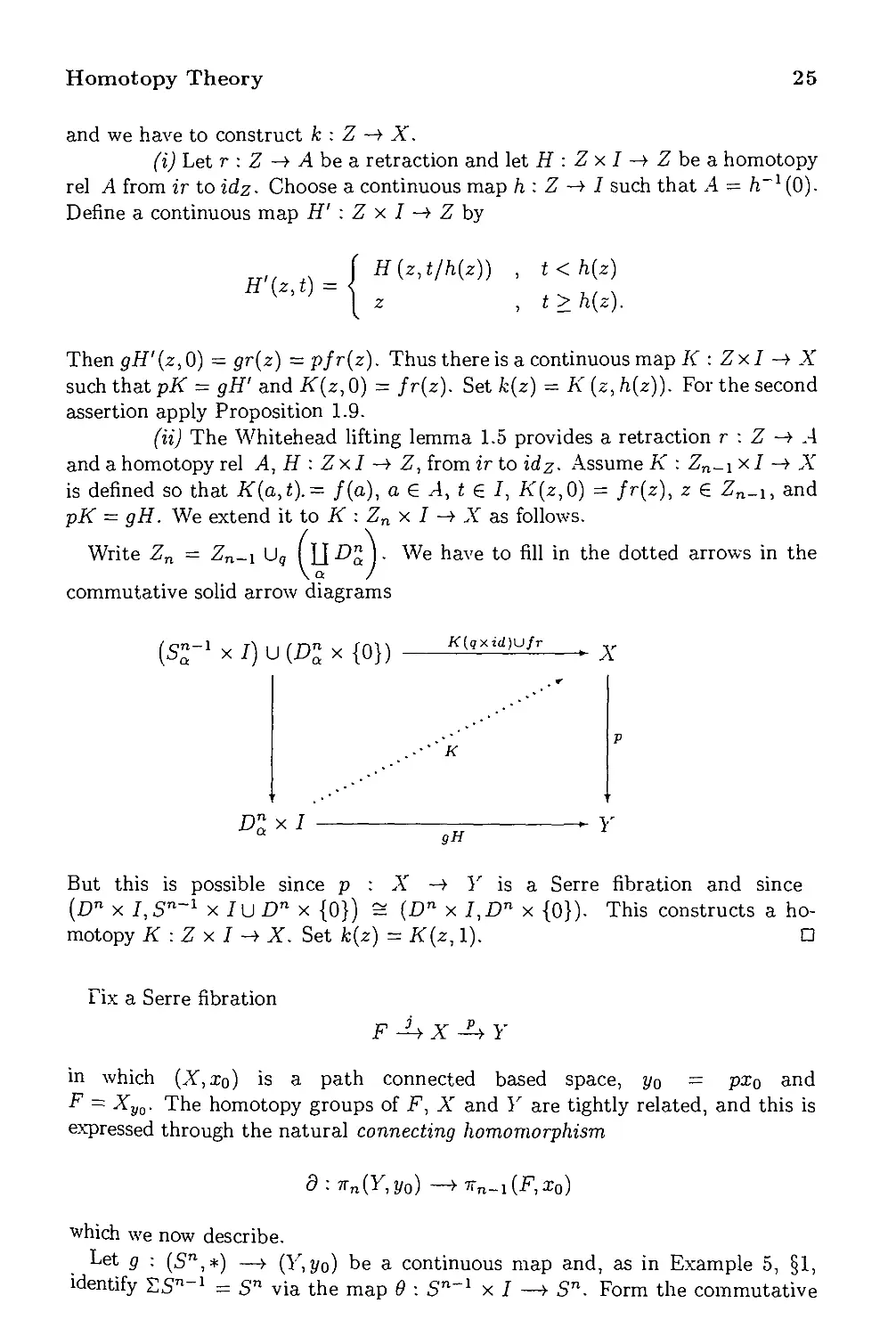
Let / : U Sq —> X be a continuous map from a disjoint union of (n — 1)
spheres S^ to a topological space X. We denote by Y = X U/ ([} D%) the
quotient space obtained from the disjoint union X]J(]J D?) by identifying ? e
52 with f(x) eX. The image el of ?? in Y is called an ?-cell and this
construction is called attaching ?-cells to X along an attaching map /. Thus
Y = X U (UQ el). The map F? : ?>? —* Y is called the characteristic map of
lU"" nM- K" ^finition it restricts to / : S" —> X.
Homotopy Theory 5
A filtered space is a topological space X together with an increasing sequence
X_1 c Xo C X\ C of closed subspaces such that
X = \J Xn and X has the weak topology determined by the Xn-
n>-\
We are now ready to introduce CW complexes, and we do so in the following
sequence of basic definitions:
• Let .4 be a topological space (necessarily a fc-space by our convention). A
relative CW complex is a pair (X, A) in which X — (J Xn is a filtered
n>-\
space, A = X-\, and there are specified identifications and maps
\aelo
and
/?'· ? S2^Xn> Xn+l = *n U/ ( ? D%+1 ) , ? > 0.
? /
?"? is called the n~skeleton of (X, A). A CW complex is a relative CW
complex of the form (?, ?).
• If X is a CW complex and X = ??, some n, then X is ?-dimensional or
finite dimensional. If X has finitely many ?-cells for each n, then A' has
finite type.
• A based CW complex is a pair (AT, Xo) with ,Y a CW complex and x0 e Xo-
• A cellular map f : (X, A) —>¦ (Y, B) between relative CW complexes is a
continuous map such that f : Xn —> Yn, n> —1.
• A subcomplex of (X, A) is a pair (Y, A) in which Y is a subspace of X that
is a union of A and of cells of X. Note that (Y,A) and (JY, 7) are then
also relative CW complexes.
We begin with some elementary remarks about the topology of CW complexes.
Let (Ar, A) be a relative CW complex with characteristic maps Fa : ?>? —>¦ Xn.
Then f : X —>¦ ? is continuous if and only if /| and / ? F? are continuous for
all ? and a.
Next, if xa G Dl - S2~l we may identify (?? - {z«}^) =
is'a~l x @,l],S?-^x {1}). Fix such an xa for each cell, and write Xn =
Xn-i U/ (\laD2), where / = {fa} : 7J 52 —> Xn_i is the attaching map.
There is then an automatic procedure for extending a subset ? C Xn-i to
V c Xn: set
6 1 CW complexes, homotopy groups and coflbrations
If ? is open in Xn-\ then V is open in Xn. Iterating this procedure ad infinitum
produces U C X such that each U ? Xk is open; i.e., U is open in X. Clearly
U ? Xn = 0, and we call U the canonical extension of 0 away from the xa.
Proposition 1.1 Let (X, A) be a relative CW complex. Then
(i) X is Hausdorff, and hence automatically a k-space.
(ii) Every compact subset of X is contained in the union of A and finitely
many cells.
(Hi) If A = 0 then X has a universal covering space.
(iv) If A is normal so is X.
proof: (i) If x,y G Xn — Xn-i (n > —1) then there are disjoint open neigh-
bourhoods O{x),O(y) C Xn — Xn-i- The canonical extensions to open sets
U(x),U(y) C X are disjoint. The general case is proved in the same way.
(ii) Let C C X be compact and choose xa G Fa (D? - SJ) so xa G C
if C ? Fa (?>2 - 52) ? 0. For each ? extend F0 (d* - S^) c Xn to an
open set Up C X, as described above and in the same way extend A =.X_i to
an open set Ua· This defines an open cover of X and xa is in Ua and in no
other Up. Since C is compact CC^U Uai U · · · U Uate. It follows that only
?
(Hi) For each ?-cell, ^?(?)^ —52) is an open subspace of
Xn — Xn-i· Extend this to an open subset U? of X away from the origins
of the other cells. It is easy to see that any loop in U? is homotopic to the
constant loop, and that the U° cover X. Hence the standard construction [68]
provides a universal cover.
(iv) Suppose C and C are disjoint closed subspaces of X. We define a
continuous function h : X —» / such that /iL· = 0 and
h\c, = 1. Indeed assume by induction that h is constructed in Xn. (Start the
induction with ? = 0 using the fact that A is normal.) If Fa : ?>?+1 —>¦ Xn+i
is the characteristic map of an (n + 1)—cell with attaching map fa : Sn —> X
define ha : F~\C U C) U 5" —> / by ha = 0 in F^iC), ha = 1 in F-l{C)
and ha = ho fa\n Sn. Since jDq+1 is normal we may extend ha to a continuous
function /?? : D?+1 ¦—> / (Tietze extension theorem [REF]). The ha extend the
construction to h ;In+1 ·—> I. D
Example 1 Complex protective space.
CPn is the space of complex lines through the origin in C"+1. S2n+l is the
unit sphere in C14. Thus assigning to ? G S2n+1 the complex line Car, we
define a map / : 52ri+1 -> CPn. The reader is invited to check that CPn+l =
CPn Of e2n+2. This exhibits CPn as a CW complex with one cell in each even
dimension 2/c, 0 < k < n. The CW complex CP°° is obtained as the union,
Homotopy Theory 7
Example 2 Wedges.
The wedge of based CW complexes, (??,??), is a CW complex whose n-
skeleton is the wedge of the n-skeleta of the Xa. ?
Example 3 Products-
Let X and Y be CW complexes. Denote the cells of X and Y respectively by
e? and /™, and let ?? : D? —> X and -? ? : D'? -> Y be the characteristic
maps for e? and /?*.
The product of the CW complexes X and Y is the space ? ? >' with the
following CW structure. The cells of dimension k are the products e? ? fjp with
? + ?? = k, and characteristic maps
DT
Dn ? Dr
? ? ?.
The attaching map of e? ? /^ is the restriction of the previous map to dDn+m =
(dDn ? Dm)U(Dn xdDm).
If X or Y is a finite CW complex then it is compact, and so the topology in
Ar ? y is the ordinary product topology. ?
Example 4 Quotients and suspensions.
The quotient X/A for a relative CW complex (X, A) is a CW complex whose
cells are the cells of X together with the additional 0-cell, [A]. In particular, the
suspension of a based CW complex is again a based CW complex. ?
Example 5 Cubes and spheres.
The unit interval is a CW complex with three cells: {0}, {1} and /. Thus the
cube /" inherits a (product) CW7 structure, and dln is a subcomplex. Note that
the CW complex In/dln coincides, as a CW complex, with the suspension of
/"-»/a/"-1.
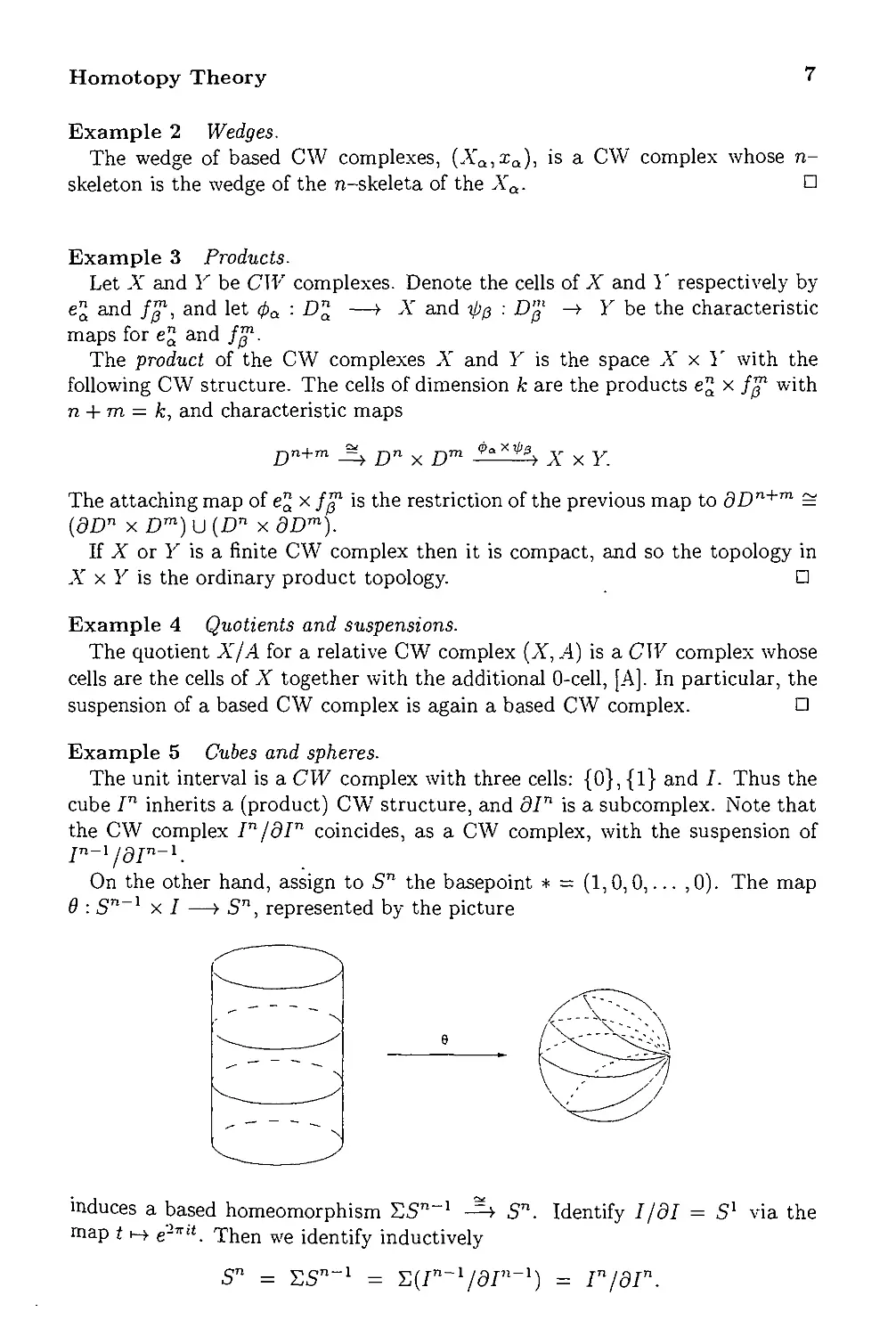
On the other hand, assign to 5" the basepoint * = A,0,0,... ,0). The map
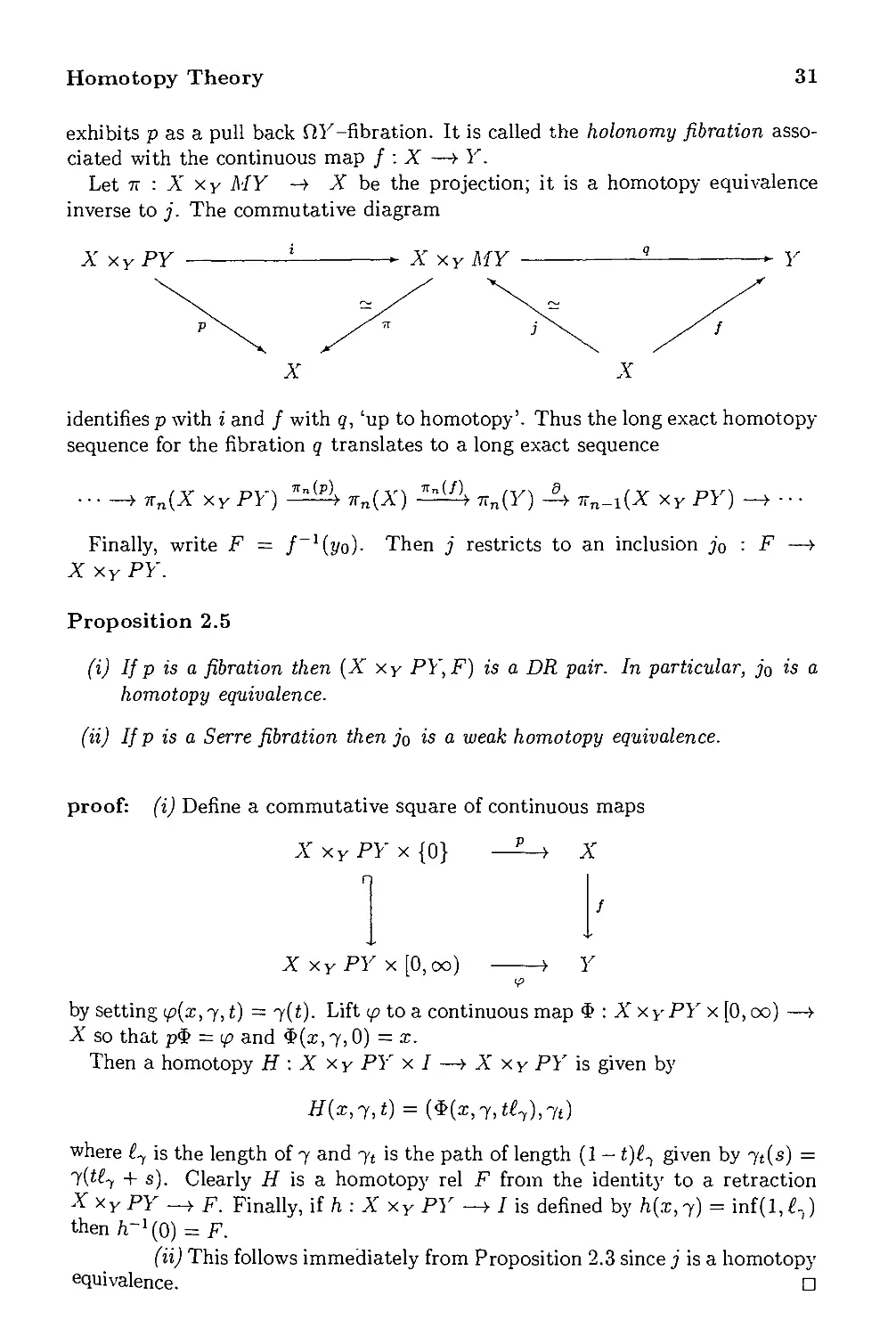
? : S" x / —y Sn, represented by the picture
induces a based homeomorphism ES" -A 5". Identify I/dl = S1 via the
map t ?» e2wit. Then we identify inductively
5" =
'1-1) = In/dln.
1 CW complexes, homotopy groups and cofibrations
This exhibits 5" as the CW complex *Uen.
There is also a homeomorphism
dP
Sn, ? > 1,
defined by translating In+1 in W+l to centre it at the origin, and then projecting
the boundary onto 5" (explicitly, ? ?-> (x — a)/\\x — a\\, where a = (\,..., |)).
The corresponding base point of dln+l is (l, |,..., |).
, |)).
?
Example 6 Adjunction spaces.
Suppose ? is a subcomplex of a CW complex ? and f : ? -> A" is a cellular
map into a third CW complex. The adjunction space X U/ ? is a CW complex
whose cells are the cells of ?' together with the cells of the relative complex
(?,?). ?
Example 7 Mapping cylinders.
Any continuous map ? : X —> Y between CW complexes may be converted
(up to homotopy) to the 'inclusion of a subcomplex' as follows: First, using
Theorem 1.2 immediately below, replace ? by a homotopic cellular map ?. Then
attach ? ? / to Y along ? ? {0} via the map ? to obtain the mapping cylinder,
? ?? ? ? /:
Since ? is cellular, ? ?_|? {? ? /) is a CW complex (Example 6). Moreover
it contains Y as a strong deformation retract (push down the cylinder) and the
inclusion of X as the subcomplex ? ? {1} is homotopic through the cylinder to
V>. ?
Next we turn to properties useful in homotopy theory. Since
(Dn xI,Dnx {0} U S71-1 ? /) S (?>" ? /, jD" x {0}), any continuous map from
Dn ? {0} U Sn'1 ? / automatically extends to Dn ? /. Now suppose {X, A) is a
relative CW complex, / : X -> Y is continuous and ?:^4?/ —»Y is a homotopy
from f\A to ?. Then (by induction on the skeleta) /U ? : ? ? {0} U ? ? 7 -» Y
extends to a homotopy X xl —t Y from / to a map g. This homotopy extension
property for relative CW complexes is important in the proof of
Homotopy Theory
9
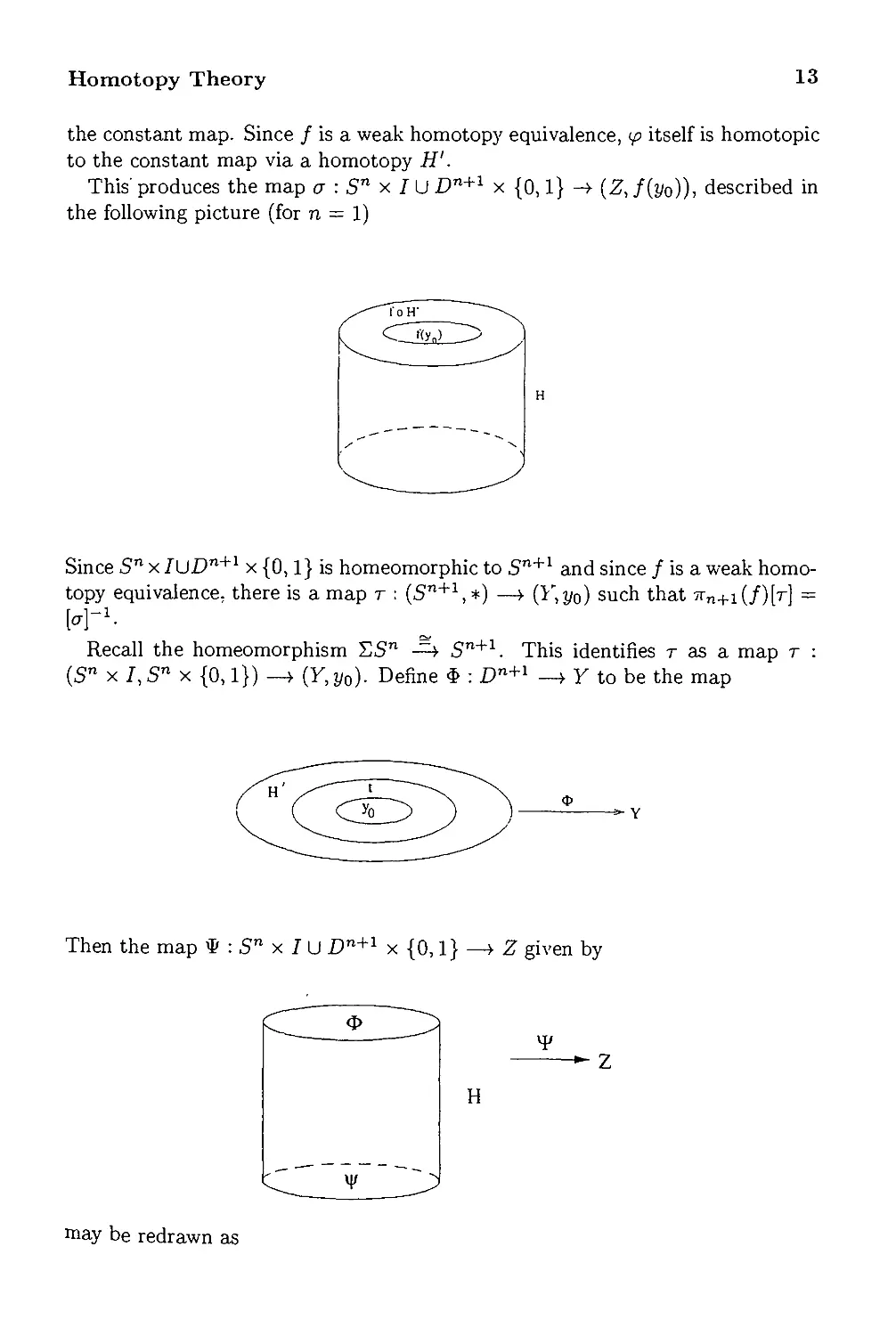
Theorem 1.2 (Cellular approximation) [160] Any continuous map
f : (X, A) —i (Y, B) between relative CW complexes is homotopic rel A to a
cellular map.
proof: Step (i) Linear approximation. Suppose ? : ? -> Rk is a continuous
map from a finite ?-dimensional CW complex. Write Zr = Zr_i U/r
and let ??,? be the origin (centre) of Dra. Any point in Dra has the form tv with
? G Sq, and 0 < t < 1. The linear approximation of ? is the linear map
? : ? -> Rk defined inductively by:
? = ? in Zo,
and
= Wfr(v) + A -
tu G
An ?-dimensional flat in Efc is a subset of the form ? + W where ? e Rk and
W C Efc is an ?-dimensional subspace. An obvious induction shows that Im#
is contained in a finite union of ?-dimensional flats. It is also contained in any
convex set C such that C D Im ?. Finally, let ? > 0 and suppose for all cells era
that \\?? — v?(orja)|| < ?, ? G era. Since
||0(it;)-y>(it;)|| < t||0/r(t;) - <pfr{v)\\ + t\\<pfr(v) - <p(or,a)\\ +
\\?(??>?) - ?(??)\\
it follows by induction that \\?? — ??\\ < 2??, ? e ?.
Step (ii) The case (X,A) = (Dn,Sn~~L). Since Dn is com-
pact, / : Dn -> Y has its image in some finite subcomplex ? D B. If dim ? < ?
there is nothing to prove. Otherwise write ? — ?' U er for some subcomplex
Z' D ? and some r > n. We construct g ~ /rel S" so that g : Dn ->
Z'U/3(Dr-{z}) for some ? in the interior of Dr. But Sr~1 is a strong deformation
retract of Dr — {z} and so Z' is a strong deformation retract of Z' Up (Dr — {z}).
Hence we can find g' : Dn -> Z' with g' ~ 5 rel 5". After finitely many steps
we have /' ~ /rel 5"-1 and /' : Dn -»¦ yn.
It remains to construct 5. For this we may as well suppose that Y = BU? Dr,
r>n. Identify (.D^S") = {In,dln). The iVth subdivision of /" is obtained
by dividing / into ?^ equal subintervals, regarding these as the 1-cells of a CW
complex structure on / and giving /" the product CW complex structure. This
is illustrated for ? = 2 by
The cells of /" will be called little cubes.
10 1 CW complexes, homotopy groups and cofibrations
By setting ||6|| = 1, b G ?, we extend the standard length function in Dr to
Y -> /. Choose ? so large that for any little cube ??, \\fxi \\ — \\fx2
<
y^, ??,?? G ??. The union of the little cubes satisfying ||/x|| > |, ? G ??
subcomplex L of/", and dln C L. Similarly the little cubes satisfying ||/rc|| < |,
? G ?? are a subcomplex K, and In = L U K.
Regard f\K as a map into the disk D C ?>r of radius |. Since ?> is convex the
linear approximation of /L is a continuous map ? : ? -i D. Choose h : Y -> /
so that /i(y) = 0 if \\y\\ > | and h{y) = 1 if ||y|| < |. Define ? : In ? / -> y by
_ ^ ? ?/?(/?)?? + A -
This is well defined because /i(/x) = 0 if a: G L. Put 5B:) = ?(?, 1).
Next recall that Im ? is contained in a finite union of ?-dimensional flats in
W. Since ? < r there is a point ? G Dr - ???? such that ||z|| < ^. If ? € ?
then ||pz|| = ||/z|| > |. If ? G A" and ?(/?) # 1 then ||/?|| > \. As"noted above
in (i), \\?? - fx\\ <ki = i- Hence II^H > H/^ll - th(fx)\\8x - fx\\.> ±. If
? ? ? and h(fx) = 1 then gx = ??. In each case gx ? ? and so ? $. Im g.
Step (in) The general case. We construct a sequence of
maps /„ : X -> Y such that /0 = /, and for ? > 1, fn(Xn-i) C Vn_i, and
In ~ fn-i rel Xn-2· Indeed given /„ we use Step (ii) in each ?-cell of X to
construct a homotopy ? rel Xn-i, fr°m fn
to a map g : Xn -> Yn. Use the
homotopy extension property to extend $ U fn : Xn ? I U ? ? {0} -? ? to a.
map ?? : ? ? I -*· y and put /n+i(z) = ??(^; 1)-
Finally, let ?? < ?? < · · · < ?? < · · · be a sequence increasing from 0 = ??
to 1. Let Hn : ? ? [??,??+?] -> Y be a map such that Hn{x,en) = fn{x),
??{?,??+?) = /n+i(z) and Hn(x,t) = /n(z), ? e Xn-i- Define ??:???->1'
by
Then ? is a homotopy rel A from / to a cellular map.
(b) Homotopy groups.
For any based space (X, xo), we write
to denote the set of based homotopy classes of continuous maps / : E", *) ->
(X,x0). When ? = 0, ???(?",??) is the set of path components of X, pointed
by the path component of x$. By convention this basepoint is denoted by 0. If
??(-?,??) = {0} then X is called path connected.
For ? > 1, ??{?,?0) is a group. Multiplication is defined via the identification
_~,_i nn ¦„ T?„nrr,n]o R ahnve; jf [f^[^ ? ???(?,?0) are represented by
Homotopy Theory 11
f,g : (ES",*) —> (?,??) then the product [/] * [g] is represented by / * g :
(ES71,*) —> {?,??), where
f(x,2t) 0<t < i ,
i <i <
The constant map c : Sn -> xo represents the identity of ??(??,??). A
continuous map ? : (X, xo) —>· (V, t/o) induces the group homomorphisms 7rn(</?) :
7r„(,Y,x0) —^ 7rn(y,y0) given by ??{?)[?] = [? ° /]; ???(^) depends only on the
based homotopy class of ?.
Definition The groups ???(?,??) are the homotopy groups of (X,xq).
If (X, *) and (Y, *) are based topological spaces, then the projections of ? ? ?
on X and on ? define group isomorphisms: ??(? ??,*) -^ ??(?, *) ? ??(?, *).
We shall often identify these groups via this isomorphism.
The identification 5" = In/dln of Example 5 identifies maps E",*) ->
(?,??) with maps {In,dln) —> (X,x0), and then
U*9Kti,...,tn) - < , . ? - .
When ? > 1 we could use the first coordinate t\ instead of tn to define a sec-
ond product, f*g. Suppose ? > 1. Then a simple check shows that there are
homotopics rel dln:
f * g ~ (f*c). * (&g) = (f*c)i(c*g)~f*g,
and
9 * / ~ (c*g) * (f*c) = (c*f)*(g*c)~f*g.
Hence ??{?,??) is abelian for ? > 2.
We recall Hopfs calculation of ni(Sn), i < n; the first assertion is a corollary
of the cellular approximation Theorem 1.2.
Theorem 1.3 [159]
(i) For i < n, ¦ni(Sn) - 0.
(ii) For ? > 1, ??E?) - ?, with 1 G ? represented by the identity map of Sn.
a
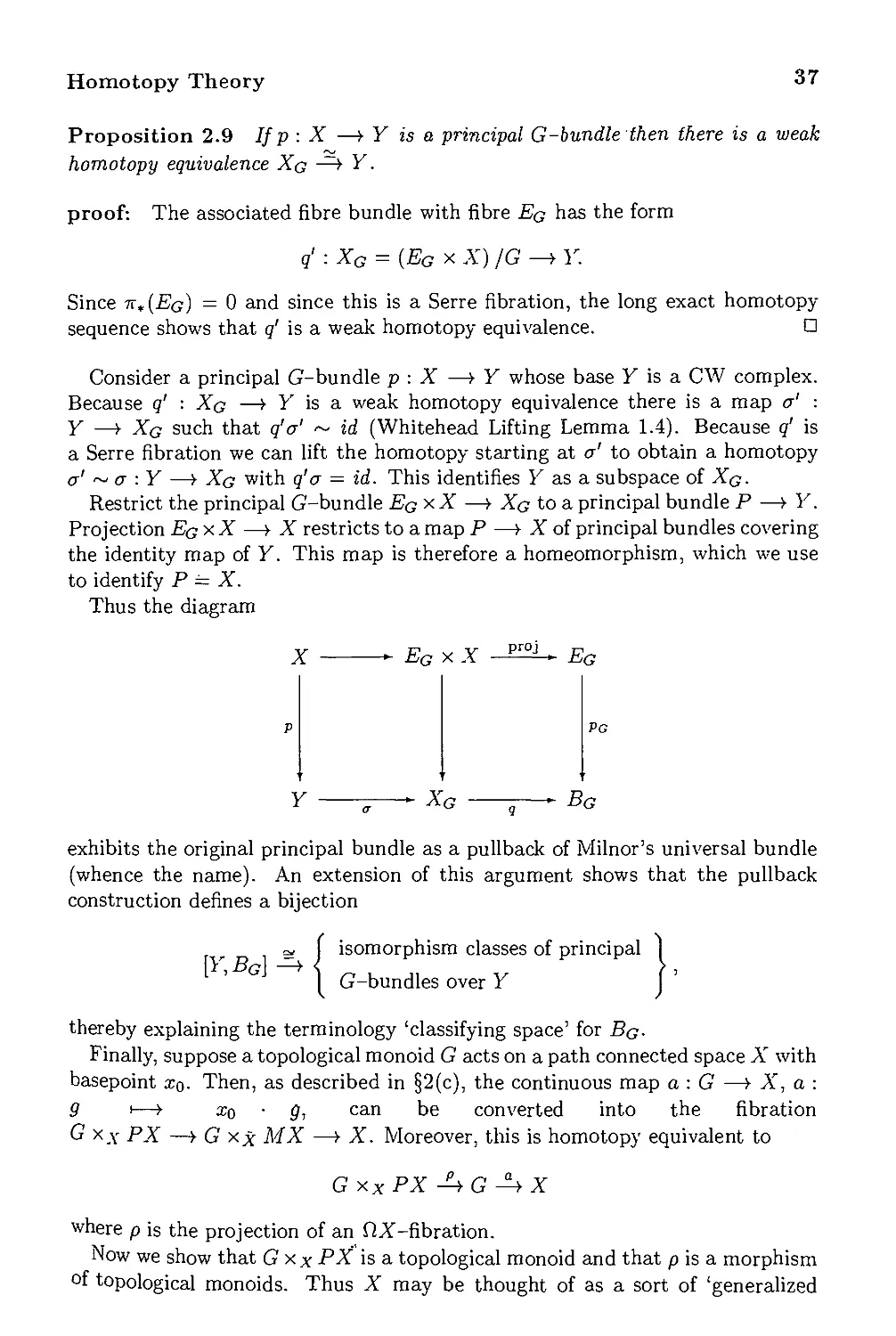
Given a path w : I -> X and a map / : (Sn,*) —> (X,w@)) we obtain a map
/ · w : (Sn, *) —4 (X,w(l)) as illustrated in the picture
collapse right hand
circles to poinis
12 ? ??? complexes, noraoiopy groups ana conorauons
This correspondence defines an isomorphism nn(X,w@)) ^4 ??(?,??A)), and
this isomorphism depends only on the homotopy class of torel {0,1}, cf. [159].
Thus if X is path connected the groups ??(?, ?), ? S X, are all isomorphic. If
they vanish for 1 < ? < r then X is r-connected (when r = 1, X is called simply
connected). When X is simply connected the isomorphisms ??(?,?) = irn(X,y)
are independent of the choice of path, and we write simply ??(?)-
(c) Weak homotopy type.
A continuous map./ : Y —> ? is a weak homotopy equivalence if ??(/) and
each
7T„(/) : *n{Y,y) —> *n(Z,f{y)), y G ?, ? > 1,
are bijections. Two spaces X and Y have the same weak homotopy type if they
are connected by a chain of weak homotopy equivalences
X 4— Z@) —>·¦¦<— Z(n) —»· y.
A cellular model for a topological space ? is a CW complex X, together with
a weak homotopy equivalence / : X —> Y.
Theorem 1.4 (Cellular models theorem) [160J
(i) Every space Y has a cellular model f : X —> Y.
(ii) If f : X' —> Y is a second cellular model then there is a homotopy equiv-
alence g : X -=> X' such that /'oj~/.
To prove this theorem one needs the fundamental
Lemma 1.5 (Whitehead lifting lemma) Suppose given a (not necessarily com-
mutative) diagram
A-*-^ Y
f
together with a with a homotopy H : Ax I —> ? from ipi to /?. Assume (X, A)
is a relative .CW complex and f is a weak homotopy equivalence.
Then ? and ? can be extended respectively to a map ? : X —> Y and a
homotopy ?: ? ? I —> ? from ? to f ? ?.
proof: As in the proof of Step (iii) in Theorem 1.2 it is enough, by induction on
the cellular structure, to consider the case that A = Xn and X = Xn+i- Then
working one cell at a time reduces us to the case that A = Sn, X = Dn+1 and i
is the standard inclusion. In this case ? : (Sn,*) —> (Y, y0) and / ? ? ~ ^1 ~
Homotopy Theory
13
the constant map. Since / is a weak homotopy equivalence, ? itself is homotopic
to the constant map via a homotopy H'.
This' produces the map ? : Sn x / U Dn+1 ? {0,1} -> (?, /(y0)), described in
the following picture (for ? = 1)
Since Sn ? IUDn+1 ? {0,1} is homeomorphic to 5n+1 and since / is a weak homo-
topy equivalence, there is a map r : (Sn+1,*) —> (l',j/o) such that ??+?(/)[?] =
[?].
Recall the homeomorphism ?5? -=> Sn+1. This identifies r as a map r :
En ? 7,5" x {0,1}) —»· (y,y0). Define ? : Dn+1 —»· y to be the map
Then the map ? : Sn ? I U Dn+1 ? {0,1} -—>· ? given by
?
?
may be redrawn as
14
1 CW complexes, homotopy groups and cofibrations
Hence ? represents [?] *[f ??] = 0, and so it extends to ? : Dn+1 ? I —> Z, as
desired. ?
Corollary 1.6 If X is a CW complex and f : Y —> ? is a weak homotopy
equivalence then composition with f induces a bisection /# : [X, Y] —> [X, Z] of
homotopy classes of maps. Similarly, if (?,??) is a pointed CW complex then
/# : [(X,xo),(Y,yo)} —»· [(X,xo),(zJ{yo))] is a bisection.
proof: The lifting theorem, applied with the relative CW complex (?,?),
shows that /# is surjective. Regard / as a CW complex with /o = {0,1}
and h — I. Then the lifting theorem applied with the relative CW complex
(? ? ?, ? ? {0,1}) shows that /# is injective. A slight variation of this argu-
ment gives the pointed case. ?
Corollary 1.7
(i) A weak homotopy equivalence between CW complexes is a homotopy equiv-
alence.
(ii) If (X, A) is a relative CW complex and A has the homotopy type of a CW
complex then X has the homotopy type of a CW complex.
proof of Theorem 1.4: First we have to show that any space Y has a cellular
model. It is sufficient to consider the case Y is path connected. We construct
f :X —*Y with X the union of subcomplexes X° C X1 C · · · C Xn C · · · and
fn the restriction of / to Xn. Fix a basepoint yo ?Y and choose /° : (X°, *) =
VQ,n S? —> {Y,yo) so that ??(/°) is surjective for all i.
Assume fn~l : I"'1 —>· Y is constructed so that also ?^/") is injective for
i < n. Since Sn = *Uen, it follows from the cellular approximation theorem that
7?? ((In-')n) —»· ???(?) is surjective. Thus we may add (n + l)-cells {e?+1}
to (Xn~1)n to kill ker7Tn (/n-1), and then extend over these cells to produce
fn : Xn = I11 U (y?e%+1^J —> Y. The cellular approximation theorem then
imDlies that ?{ (/") is injective for i < n.
Homotopy Theory 15
The uniqueness (up to homotopy) of a cellular model follows at once from the
Whitehead lifting lemma and its corollaries. ?
(d) Cofibrations and NDR pairs.
Suppose A is a subspace of a topological space ?'. The pair (X,A) is a
cofibration if for any continuous map f : X —> ? ? homotopy ? : A x I —> Y
starting at f\A always extends to a homotopy ? ? I —> Y starting at /.
Lemma 1.8 (A', A) is a cofibration if and only if ? ? {0} U A x I is a retract
of ? ? I.
proof: A continuous map / : X —> Y and a homotopy H : A x I starting at
f\A define a map (/, H) : X x {0} U ? ? I —>· Y. If r : ? ? I —»· ? ? {0} UA ? I is
a retraction then (/, H) or : ? ? I —> Y extends H and starts at /. Conversely,
if (A", A) is a cofibration take Y = ? ? {0} U Ax I and (/, ?) the identity map.
An extension of ? starting at / is a retraction r : ? ? I —> ? ? {0} U ? ? ?.?
Again suppose A is a subspace of a topological space X.
Definition (i) The pair (X, A) is a DR pair if A is a strong deformation retract
of X and A = h'1 @) for some continuous function h : X —> I.
(ii) The pair (A, A) is an NDR pair if for some open U C X there
are continuous maps H :U ? I —> X and h : X —> I such that:
• A = ?-^?) and U D h-l([0,e)), some ? > 0.
• ? is a homotopy rel A from the inclusion of U in X to a retraction U —> A.
(iii) A well-based space is a an NDR pair (X,x0).
Proposition 1.9
(i) If (A, A) and (Y, B) are NDR pairs then (? ? ?, ? ? ? U ? ? ?) is an
NDR pair.
(ii) A relative CW complex (A, A) is an NDR pair.
proof: (i) Let H, h, U be as in the definition for the NDR pair (A, A) and let
A,fc, V be analogous maps and open set for (Y, B). Define ? : ? ? ? —> I by
t{x,y) = inf (h{x),k(y)). Choose a continuous function a : (?, [?,?/2], [?, 1]) -—>·
(? 1,0) and define ?' : ? ? I —»· ?, ?' : Y x I —»· Y by
??? ? - S H(x,a(hx)t) , x
{ ' ' ~ \ ? , h
hx > ?
and
16 1 CW complexes, homotopy groups and cofibrations
Ky ' \ y , ky > ? .
Define L:A'x7xMArx Y by
({^t),K'(y,t)) , k(y)<h(x)
L(x.,y,t)=l ))
Set ?/' = h'1 ([0,?/2)), V = k'1 ([0,?/2)) and W = U'xYuXx V. Then
L, t, W exhibit (?' ? ?, ? ? YuX ? ?) as an NDR pair (where we use ?/2 instead
???).
(it) Define a continuous function h : X —> I by induction on the skeleta,
as follows. Set h = 0 in A. If h is defined in Xn~i and /Q : 5" —>· X„_i is
the attaching map of an n-cell ?>" then let || || be the length function in ?>?
and extend h to Xn by
Then A = /i-1@) and ? ([0,1/4]) is contained in the canonical extension of A
to an open set U in X (away from the origins oa of the cells ?>").
We show now that A is a strong deformation retract of U. Put
Un = U ? Xn, and note that t/0 = A. Define a retraction rn : Un —> Un-i
by rn{x) = /?(?/||?||), ? S UHD2, and define Kn : Un ? I —> Un by
JiTntz, i) = A - t + t/\\x\\) ?., ? G U ? D^. Thus ??? exhibits C/n_i as a strong
deformation retract of Un.
Put rfc_n = rfc ? · · · ? rn : Un —> Uk-i· Then a retraction r : U —> A is
defined by rx = ri>n(x), ? G Un. Set 7n = hj^y, ^ and turn ATn into a map
iin : Un ? In —> Un via the obvious map Un,;^,^) —> G,0,1). Define
H : U ? / —> U by setting (for ? G f/n)
[ iffc (rfc+1>n(z),i) , t G 4, 1 < k < ? .
Then ? exhibits A as a strong deformation retract of U. ?
It turns out that the condition ?NDR pair' is only slightly stronger than the
condition 'cofibration'.
Proposition 1.10 The following conditions are equivalent on a topological pair
Homotopy Theory 17
(i) A is closed in X and (X, A) is a cofibration.
(ii) (X, A) is an NDR pair,
(in) (X x 7, X x {0} U A x 7) is a DR pair.
proof: (i) => (ii): Let r = (rx, 77) : ? ? I —> X x{0}uAx I be ? retraction
(Lemma 1.8). Define h : X —»· 7 by ?(?) = sup {i-r/(z,i) | t G 7}. Set
[/ = /i-1 ([0,1)), and set H = rx : U ? 7 —»· X.
(mJ => CinJ; Let h,U,e and ii be as in the definition of NDR pairs.
Choose a continuous function ? : (/, [0, ?/2], [?, 1]) —> [1,1,0) and set ? = ah :
X —> 7. Define A':Xx/-^Iby
? 7i(z,(y)z)i) , xGI/
K{x,t) = <
\ ? , hx > ? .
Define A; : X —»· 7 by fcx = inf (fAz,l). Then ? : Ar1 ([0,1)) x {1} —»· ^4·
Define a homotopy ? : (? ? 7) ? 7 —>· ? ? 7 by
, t < kx
{ (K(x,s), t-skx) , t>kx.
It is straightforward to see that ? is a homotopy rel ? ? {0} U A x I from idxxi
to a retraction onto ? ? {0} U A x I.
(in) =$> (i); This is Lemma 1.8. ?
Proposition 1.11 Suppose {X,A) is an NDR pair. Then the inclusion i :
A —> X is a homotopy equivalence if and only if (X,A) is a DR pair. In this
case (? ? ?,? ? {0,1} U A x 7) is a DR pair.
proof: If (X, A) is a DR pair then i is certainly a homotopy equivalence. Sup-
pose that i is a homotopy equivalence. Choose ? : X —^ A so that ?? ~ id a·
Extend the homotopy to a homotopy ? ? I —> A from ? to a map r : X —> A.
Clearly ri = id a- Now, since i is a homotopy equivalence, there is a homotopy
? : ? ? I —> X from idx to ir. Define .FT : X xIx{0}uX x{l}xIUAxIxI -^
X by setting
and
H(irx,2-2t) , \ <t < 1 ,
= irx ,
Ji(o,2(l-5)i) , 0<i<i
K(a,t,s) = < ,
It is easy to construct a homeomorphism
18
1 CW complexes, homotopy groups and cofibrations
(/x/,/x {O}U{1} ?/) {???,?:
Thus {? ? ? ? 7, ?" ? ? ? {0} U ? ? {1} ? ? U ? ? 7 ? 7) S (? ? 7 ? 7, ?" ?
7 ? {0} U ? ? 7 ? 7), which is a DR pair by Proposition 1.10. In particular K
extends to a map ? : ? ? ? ? I —> X, and K(x, t, 1) is a homotopy rel A from
idx to ir. Thus (X, A) is a DR pair.
Finally, since (X, A) is a DR pair, clearly A x {1} and A x {0} are strong
deformation retracts of ? ? {0} and X x{l}, and so A x I is a strong deformation
retract of ?' ? {0,1} U A x 7. Since the composite Ax I —> ? ? {0,1} UA x 7 —>
? ? I is also a homotopy equivalence it follows that the inclusion ? ? {0,1} U A x
I —> ? ? I is a homotopy equivalence as well. Thus (? ? 7, ? ? {0,1} U A x 7)
is a DR pair by the first assertion of the proposition. ?
(e) Adjunction spaces.
If (X,A) is an NDR pair and f : A —> Y is a continuous map then it is
immediate that (Y \Jf X, Y) is also an NDR pair. (Note that a continuous
function h : X —> I with /i-1@) = A can be used to separate the points of Y
from the points of X — .4, so Y U/ X is Hausdorff.)
Lemma 1.12 If (X, A) is an NDR pair and /o,/i : A
continuous maps then Y U/o X — Y Uf1 X.
Y are homotopic
proof: Choose a homotopy H : Axl —> Y from j? to f\. DenoteXx{i}UAx7
by Bt C X x I. Proposition 1.10 implies that each Bt is a strong deformation
retract of ?" ? 7. Hence Y Uh Bo and Y Uh ?? are strong deformation retracts
of Y UH (? ? 7). But Y Uh Bo = 1' U/o A and Y \JH ?? = Y Ufl A. D
Next, consider a commutative diagram
y ~* -?. *" ji.
of continuous maps.
Theorem 1.13 If i and i' are the inclusions of NDR pairs and ?$??,?? an
?? are homotopy equivalences then
? = (??,??) ¦ Y U/ X —> Y' ur X'
Homotopy Theory 19
is a homotopy equivalence too.
The proof will rely frequently on the following obvious remark.
Lemma 1.14 If(B, C) is a DR pair and h : D —> W is a continuous map from
a closed subspace D C C then (W Uh B, W U^ C) is a DR pair. In particular,
the inclusion
W Uh C <^-> W \Jh ?
is a homotopy equivalence. ?
proof of Theorem 1.13: Identify (A',.4) with (X,A) ? {1} C X x 7. Then
(? ? I, X) is a DR pair (trivially) and {? ? ?, ? ? {0} U A x 7) is a DR pair by
Proposition 1.10. Moreover / is identified as a map / : A x {1} —> Y and by
Lemma 1.14,
Y U/ (X x {0} U A x 7) —> V U/ (? ? /) f- ? U/ X
are homotopy equivalences.
Denote X \J Y by ? and </?? JJ </?y by </?z, and define g : A x {0,1} —> ? by
p(o,0) = io and g{a, 1) = /a. Then Y Uf (? ? {0} U.4x7) = Z Us (.4 ? I). It
is thus sufficient to show that
(??,?? x id) : ? Us {A x I) —> Z' Uff- (.4' ? 7)
is a homotopy equivalence. Since this map factors as
? Up {A x I) —> Z' Uvzg {A x I) —> Z' LV (-4' x /)
it is sufficient to consider the two special cases: either A = A1 and ? a — id or
else ? — ?' and ? ? = id.
Case 1: ? = ?' and ?? = id:
Regard ? a as a map ? ? {0} —> A' and set ? = ?' ??? (? ? I). Define a
retraction r : ? —> A' by r(a, t) — </>>?<2, and set
gB = g> ? (r x ii/) : ? x {0,1} —> ? .
If C c ? let 5c : C x {0,1} —>· Z be the restriction of g ?. Thus the inclusion
of C in ? and the retraction r define an inclusion and retraction
? Usc (Cxi) ^ ? UgB (???)?? Uff. (.4' ? 7) .
Now suppose {B, C) is a DR pair. Then so is {? ? ?, ? ? {0,1} U C x 7), by
Proposition .1.11. Since ? USc (C ? 7) = Z UgB (B x {0,1} U C x 7) it follows
triat je is a homotopy equivalence (Lemma 1.14). In particular {B, A') is a
DR pair and, clearly, qja1 — id. Thus ? is a homotopy equhralence. Moreo\rer,
if we identify A = A x {1} C B then the inclusion is a homotopy equivalence
20 1 CW complexes, homotopy groups and cofibrations
because ? a is. Thus (B,A) is a DR pair (Proposition 1.11) and Ja is a homotopy
equivalence.
Finally, (???,?? x id) = QJ.a and so it is a homotopy equivalence too.
Case 2: A = A' and ? a = id:
Denote (AxI, Ax{0,1}) by (B,C). Thus g : C —> ? and we have to show that
if ?? : ? —> ?' is a homotopy equivalence so is (??, id) : ZUgB —> ?' ???9 ?.
Suppose first that ? — ?' and id ? ~ ?? via a homotopy ? : ? ? ? —> ?. Set
? = ? ? (g ? id) : C ? ? —> ? and set
? = (?, idBxi) ¦¦ (ZUg ?) ? I —> ? \JH (? ? I) .
In the proof of Lemma 1.12 we observed that the inclusions b ?—> (?,?) and b ?—>
(b, 1) of ? in ? ? I induce homotopy equivalences j0 : ? \Jg ? —> ? U# (B x I)
and ji : ? ???9 ? —> ? U// (? ? I). A quick check shows that ? is a homotopy
from jo to ji ? (ipziid). Hence (? ?-.id) is a homotopy equivalence.
Now suppose ?? is any homotopy equivalence and choose a homotopy inverse
?? '¦ ?' —> ? : ???? ~ id ?1 and ???? ~ id z- Form the sequence
? \Jg ? —> ? Upzs ? —> ? ^¦????9 ? —> ? {->??????9 ? ,
where 70 = (? ? Ad), 7? = {'? ?, id) and 72 = (? ?, id).
The argument above shows that 7170 and 7271 are both homotopy equh'a-
lences; i.e., they have homotopy inverses a and ?. Thus ??? ~ /572717??: ~ 7ou,
and so 7i(/5727i7ou) ~ l\?li ~ 7i7ou ~ id ? and (/5727i7ouOi ~ idz· · Thus
71 is a homotopy equivalence. Hence so is 70 = (??, id). ?
Corollary If (X, A) is an NDR pair and A is contractible then the quotient
map q : X —> XjA is a homotopy equivalence.
proof: Identify q as the map (c, id) : A\JaA X —> pt Uc X, where c : A —> pt,
and note that c is a homotopy equivalence by hypothesis. ?
(f) Cones, suspensions, joins and smashes.
The cone, CX, on a topological space X is the space ? ? IjX ? {0}, and the
point [? ? {0}] is· called the cone point. We usually denote the point (x,t) by
tx, so that Ox is the cone point (any ? ? X). This identifies X as the subspace
X x {1} and clearly (CX,X) is an NDR pair.
Recall that the suspension of a based topological space (X, xq) is the based
space
?? = (?? ?)/? ? {0,1} U {x0} xl = CX/X U {x0} x I .
Any continuous map / : (X, x0) —> (Y, yo) suspends to the map ?/ : ?? —> ??
induced from / ? idi. Note that if (X, x0) is well based then (? ? ?, ? ? {0.1}U
(??\ ? I) is an NDR pair (Proposition 1.9). Hence ?? is well based. Moreover, if
Homotopy Theory 21
(F, y0) is also well based then Theorem 1.13 implies that homotopy equivalences
suspend to homotopy equivalences.
The join of two topological spaces X and F is the subspace ?' * Y C CX x CY
of points of the form (tx, A - t)y), tel. (Thus X * Y is the union of intervals
joining ? ? X to y 6 Y.) Notice that a homeomorphism
{CX x F) UXxy (? ? CY) -=> X * Y A.15)
is given by (iz,y) ?—> (far, (l- f) y) and (z,iy) ?—> ((l- f ) ?, |y).
More generally, the ?-fold join ?? * · · · * Xn of topological spaces X; is the
subspace of CX\ ? · · · x CXn of points (iiXi,.... inxn) satisfying ??; = 1. Note
the ob\dous identifications
(?? * X2) * Xz = Xi * X-2 * X3 = Xi * (X2 * X3) ·
Next, recall that the wedge X V Y of based spaces (X,xq) and (V, yo) is the
subspace X x {yo} U {^o} x F of ?' ? F. The smash product of A" and F is the
space
? ? F = ? ? ?/? V ? .
In particular ?? coincides with ? ? S1 and so
XAEF^XAFAS1 = ?(? ? F) . A.16)
Proposition 1.17 // (X,x0) o,nd (F, yo) are well based spaces then there is a
homotopy equivalence, X * F —t ?(? ? F).
proof: Embed / as Ix0 and Iyo in CX and CY. Identify CX U/ CF as the
subspace of X * F of points (tx, A — t)y) such that either a; = x0 or y = yo· Now
(CX U/ CF)/J = (CX/J) V (CF/J). Since I is contractible we can apply the
Corollary to Theorem 1.13 to obtain
CX U/ CY ~ (CX U/ CF) // ~ CX V CY ~ {pi} .
Hence (by the same Corollary) the quotient map X * F —> X * Y/CX U/ CF is
a homotopy equivalence.
But if q : X x F ? ? —> ? * F is the quotient map (x, y, t) ?—>· (tx, A - i)y)
then q-1 (CX U/ CF) is just (X V F) ? I. Hence c induces a homeomorphism
?(? ? F) -=> ? * F/CX U/ CF. D
Exercises
!· Prove that F(X,x0) is contractible and that X and Xi0'1) have the same
homotopy type.
22 1 CW complexes, homotopy groups and cofibrations
2. Let ?, ? and ? be based spaces. Prove that (XvY)aZ =* (XAZ) V(V ??),
(? ? y) V ? = (? V ?) ? (y V ?) and (? ? y) ? ? ~ ? ? (y ? ?).
3. Prove that ? * y is homeomorphic to the inverse image of the set {(s.,t) 6
[0,1]2 ,5 + t = 1} under the mapping CX ? CX -» [0.. If, ([z,s], [y,i]) ^ (s,i).
4. By considering the mapping Em+1 ? ??+1 ? [0,1] -> Em+n+2 ,{x,y,t) i->
(x cos ^, y sin f ), prove that 5n * Sm = 5m+n+1.
5. Let / : S3 -> CP1 = S2 be the map defined by f{zuz2) = [21,23]· Prove
that there exists a homeomorphism from the cofibre of / onto CP2.
6. Let (?,??) and (Y,yo) be based spaces. Prove that the inclusion ?' V
y c—? ? ? I' is a cofibration whose cofibre has the same homotopy type as
? ? ? and that ?(? ? y) has the same homotopy type as ??' V ?? ??(?'? ?).
2 Fibrations and topological monoids
A continuous map ? : X ->· Y has the lifting property with respect to a pair of
topological spaces (Z. A) if for every commutative diagram of the form
.4 -J-+ X
9
there exists a continuous map k : ? -^ X such that pk = g and ki = f.
A surjective continuous map ? : X -» Y is a 5erre fibration if it has the lifting
property with respect to (? ? ?, ? ? {0}) for all CW complexes Z, and a fibration
if it has the lifting property with respect to (? ? ?, ? ? {0}) for all topological
spaces Z. Here ?' is called the total space, ? the projection and Y the base of
the fibration. The space Xy = p~xB/) C X is called the fibre at y. If Y has a
fixed basepoint y0 we often denote the fibre at y0 by F and denote the (Serre)
fibration by F -> X A Y.
A fibre-preserving map between (Serre) fibrations is a commutative square of
continuous maps
X' —j~^ X
Y'
Y
in which p' and ? are (Serre) fibrations.
In this section we first establish a long exact sequence connecting the homotopy
groups of the fibre, total and base spaces of a fibration. Next, we construct the
Moore loop space UX of a based topological space (X,*). This is a topological
monoid acting on the contractible path space, PX, which identifies UX as the
fibre of the path space fibration PX —> X.
This construction generalizes. Given a continuous map / : X —> Y there is a
homotopy equivalence Xxy MY -^» X which converts / into a fibration whose
fibre Xxy PY admits a natural action of ??. The projection ? ? ? ?? —> X is
then also a fibration (the holonomy fibration) with fibre ?? given by the action.
Finally, we consider principal bundles with fibre a topological group and con-
struct their classifying spaces.
This section is organized into the following topics:
(a) Fibrations .
(b) Topological monoids and G-fibrations.
(c) The homotopy fibre and the holonomy action.
24 2 Fibrations and topological monoids
(d) Fibre bundles and principal bundles.
(e) Associated bundles, classifying spaces, the Borel construction and the
holonomy fibration.
(a) Fibrations. We begin with some examples.
Example 1 Products.
The projection F x F —> Y is a fibration (the trivial fibration). ?
Example 2 Covering projections.
These are fibrations [68]. ?
Example 3 Fibre products and pullbacks.
Given continuous maps A —> Y ^- X, recall that the fibre product A xy X C
A x X is the subspace of pairs {a,x) such that f(a) = p(x). It fits into the
commutative pullback diagram
??? ?
A
X
Y
in which ? a and g are projection on the first and second factors. If ? : X -» Y is
a (Serre) fibration then so is ? a '· A x ? ? -» .4. It is called the pullback fibration.
When / : A -» Y is the inclusion of a subspace then A x y ? = ? (.4) and
the fibration ? a is called the restriction of the original fibration to A. ?
Proposition 2.1 (i) A fibration ? : X -» Y has the lifting property with respect
to any DR pair (Z,A). In particular, if (W,B) is any NDR pair then ? has the
lifting property with respect to (W ? I, IV x {0} Li ? ? I).
(ii) A Serre fibration ? : X -» Y has the lifting property with
respect to any relative CW complex (Z, A) for which the inclusion A -t ? is a
weak homotopy equivalence. In particular, if{W,B) is any relative CW complex
then ? has the lifting property with respect to (W ? I, W ? {0} Li ? ? I).
proof: [157] In both cases we suppose given a commutathre square
Y
Homotopy Theory
25
and we have to construct k : ? -> X.
(i) Let r : ? -> A be a retraction and let iT : ? ? ? -> ? be a homotopy
rel A from ir to idz- Choose a continuous map h : ? -> I such that A = h'1 @).
Define a continuous map if' : ? ? ? —? ? by
H'{z,t) =
H{z,t/h{z)) , t<h(z)
? , t > h{z).
Then gH1 (z,0) = gr(z) = pfr(z). Thus there is a continuous map ? : Zxl -? ?
such that pK = gH' and K(z, 0) = /?). Set k(z) = ? (?, /?(?)). For the second
assertion apply Proposition 1.9.
(ii) The Whitehead lifting lemma 1.5 provides a retraction r : ? -» ?
and a homotopy rel ?, ? : ? ? I -» ?, from ir to idz· Assume iv : ??_? ? 7 -> JV
is defined so that K{a,t).= /(a), a ? A, t ? I, K(z,0) = /r(z), ? ? Zn-U and
pii = gH. We extend it to ? : Zn ? I -> ? as follows.
We have to fill in the dotted arrows in the
Klqxid)\Jfr
Write Zn = Zn_! U9
commutative solid arrow diagrams
(S2'1 ? 7) U {Dl x {0})
Z ? ?
Y
But this is possible since ? : ? —» ? is a Serre fibration and since
{Dn ? 7,5?-1 x/UU"x {0}) = (?>n ? I,Dn ? {0})· This constructs a ho-
motopy ? : ? ? I -> X. Set fc(z) = AT (?, 1). D
Fix a Serre fibration
X
in which (X,Xq) is a path connected based space, y0 = pxc, and
F = Xyo. The homotopy groups of F, ?' and Y are tightly related, and this is
expressed through the natural connecting homomorphism
which we now describe.
Let g : En,*) —> (Y,yo) be a continuous map and, as in Example 5, §1,
identify ?5"-1 = 5" via the map ? : S" ? ? —> Sn. Form the commutative
26
diagram
2 Fibrations and topological monoids
S" X {0} U {*} X I
constant map to
?
x I
sn
Y
Since ? is a Serre fibration, Proposition 2.1 asserts that gQ can be lifted through
? to a continuous map ? : Sn~l x / —> X, which then restricts to a map
Proposition 2.2 [93] With the notation above the correspondence g ~* h de-
fines natural set maps dn : ??(?,yo) —> nn^i(F,xQ). When ? > 2, i/iese are
group homomorphisms, and fit into a long exact sequence (the long exact homo-
topy sequence)
When ? = 1, 9? : 7??(?)
factors to give a bijection
proof: Put W = 5n ? J and ? = 5n ? {0} U {*} ? I. Then the inclusion
W x {0,l}U?x/ C—>· W x I is a weak homotopy equivalence. Hence it follows
from Proposition 2.1(ii) that the based homotopy class of h is independent of
the choice of H and depends only on the based homotopy class of g.
Recall from §1 that maps (Sn,*) —> (X,Xq) may be identified with maps
(In,dln) —> (X,x0), and that, when ? > 1, composition along the first coor-
dinate defines a second product, fig, such that fig ~ / * g rel dln. But it is
immediate from the definition that when ? > 2, dn[f*g] = [dnf]*[dng]\ hence
<9n is a group homomorphism.
The exactness of the sequence and the assertion about d\ are straight forward
consequences of the definition and Proposition 2.1(ii). D
Next let
A Xy X
X
Y
Homotopy Theory 27
be a pullback diagram (i.e., q and gx are the projections on ,4 and X). Recall
that if g is the inclusion of a subspace .4 C Y then gx is the inclusion of
Proposition 2.3 Suppose in the diagram above that ? is a fibration.
(i) If g is the inclusion of a DR pair (resp. an NDR pair), (Y, A) then (X, XA)
is also a DR pair (resp. on NDR pair).
(ii) If g is a homotopy equivalence so is gx.
proof: (i) Suppose (Y,A) is a DR pair. Let H :Y x / —> Y be a homotopy
rel A from idy to a retraction r : Y —> A. Let h : Y —> I be a continuous
function such that h~l@) = A and define a homotopy H' : X x I —> Y by
j H(px,t/h(px)) , t<h(px) .
? (x.t) = < . v
v ; | px , t> h(px) .
Lift this to a homotopy K' : ? ? J —>· X starting at id ?. Define ? : X xl —> X
by
? ??(?,?) , ?</?(??) .
(X' j \ K'{x,h{px)) , t>h{px) .
Then ? exhibits X^ as a strong deformation retract of X. Thus ? together
with k = hop exhibits (X,Xa) as a DR pair.
An identical argument shows that if {Y,A) is an NDR pair then so is (X,Xa)·
(ii) Regard g as a map ? ? {0} —> Y, and let r : (? ? J) Us Y —> Y
be the retraction sending (a, t) to ga. Denote (A x /) Us Y by ? and form the
pullback diagram
ZXyX —?±-+ ?
Now identify A = A x {1} C Z. Then the inclusion of .4 in ? is a homotopy
equivalence, because g is, while the inclusion of Y in ? is obviously a homotopy
equivalence. Since (Z,A) and (Z,l') are clearly NDR pairs, Proposition 1.11
asserts they are NR pairs. Now assertion (i) of this proposition implies that
(? ???,? xyX) and (? ???,? ?? X) are DR pairs. But Y xy X = X and
?? ·. ? ?? ? —>. ? is a ]eft inverse for the inclusion. Hence ? ? is a homotopy
equivalence. Moreover, gx factors as A x y ? —> ? ? y X -^ A", and so it is a
homotopy equivalence too. ?
28 2 Fibrations and topological monoids
Finally, let
be a fibre preserving map between Serre fibrations with path connected total
spaces. Then from Proposition 2.2 and the five lemma (see Lemma 3.1) we
deduce
Proposition 2.4 Let y' € Y' be any basepoint. If any two of the maps f, g
and fy> : X', —\ Xgy> are weak homotopy equivalences, then so is the third. ?
(b) Topological monoids and G-fibrations.
A topological monoid is a topological space G equipped with a continuous,
associative multiplication ? : G x G —> G and a two sided identity e € G. A
(right) action of G on a space ? is a continuous map ? ? G —> ?, (?, g) *-> ? ¦ g,
such that ? ¦ e = ? and (? ¦ g\) · gi = ? · (gig?), for ? € ? and g,g\-,g2 ? G.
Suppose ?' ? G' —> P' is a right action of a second topological monoid G'.
Then an equivariant map consists of a morphism 7 : G —> G' of topological
monoids and a continuous map / : X —> X' such that /(?·?) = f(x) -7@).
If / and 7 are weak homotopy equivalences then (/, 7) is called an equivariant
weak equivalence.
Definition A G-(Serre) fibration is a (Serre) fibration ? : ? —y X together
with a right action ? ? G —> ? such that
(i) p(z ¦ g) = pz, ? G P, g G G, and
(ii) For each ? G ? the map Az : G —> Ppz sending g t-> ? ¦ g is a weak
homotopy equivalence.
Remark 1 If ? is path connected and some Az is a weak homotopy equivalence
then so is every Aw, u> € P. Indeed, join ? and u> by a path ? : I —> P. Then
we have a map of fibrations
I xG
/
, f(t,g) = (?,?(?) -g).
/
Let ft be the restriction of / to the fibres at t. Then Proposition 2.3 asserts that
/o is a weak homotopy equivalence ¦$=$> f is a weak homotopy equivalence
<?=^ fi is a weak homotopy equivalence
Homotopy Theory 29
But /o = Az and /i = Aw.
Remark 2 If / : Y —> X is a continuous map the pullback ? ? ? ? —> ? of a
G- (Serre) fibration is again a G-(Serre) fibration with action (y, z)-g = (y, zg),
y€Y,z€P,g€G.
Example 1 Path space fibrations.
As usual, XY denotes the space of all continuous maps Y -> X. Injparticular,
let P(X,x0) C X1 be the subspace of paths ending at xo, and let Cl(X,x0) C
P(X,:ro) be the subspace of paths beginning and ending at xo· Then it follows
easily from the exponential law that
?(?',??) > P(X,xo) —^ ? , ? : 7 —> 7@),
is a fibration, and that PX is contractible. Note also that a continuous multi-
plication in QX is defined by
f 7Bi) 0 < t < i
G,?) ?—»7*?, G*?)(?) = < , ,
?> ' ' w ;w [ ?B?-1) I <i < 1 .
This is 'homotopy associative', but not associative. These constructions are the
classical version of the path space fibration and the loop space for X. It turns
out to be highly convenient to use instead Moore's version of the path space
fibration and loop space, since the latter is a genuine topological monoid.
Thus a Moore path in X is a pair G, ?) in which 7 : [0,00) -> X is a continuous
map, i € [0,oo), and j(t) = ?(?) for t > L The path starts at 7@), ends at
j(?) and has length t. The space MX C Xt0·00) ? [0, oo) of all Moore paths is
the free Moore path space on X. The Moore path space P(X,xo) C MX is the
subspace of all Moore paths ending at Xq and the Moore loop space ?(?,??) is
the subspace of all Moore paths starting and ending at xo· As above, we usually
abuse notation and write simply QX and PX. We may also abuse notation and
denote G, ?) simply by 7. From the exponential law it follows easily [157] that
if ?" is path connected then
UX —* PX —^-> X pG, t) = 7@)
is a fibration and that PX is contractible. This is called the Moore path space
fibration for (X,x0). Note that QX and PX can be identified as the subspaces
of ?.? and PX of paths of length one, and that these inclusions are homotopy
equivalences.
If 7 € PX and ? € ?? are paths of lengths ? and r we define their product
to be the path 7 * ? of length ? + r given by
7@, 0<i<^
30 2 Fibrations and topological monoids
This product, restricted to QX ? QX, makes QX into a topological monoid with
identity the constant loop of length 0 at zo· It is called the Moore loop space of
X at xo·
The original map, PX ? QX —> PX is a continuous right action of the monoid
QX on the contractible space PX. Note that p(j * ?) = py, 7 e PX, ? 6 QX.
Moreover ??' is the fibre (PX)Xo and the map Ae : QX —> (PX)Xo is just the
identity, and so a weak homotopy equivalence. Thus it follows from Remark 1
that the Moore path space fibration PX —> X is an ??-fibration.
We shall often abuse language and refer to PX and QX simply as the path
space and loop space of X. Finally, if / : (?',??) —* (Y, 2/o) is any continuous
map then ?/ : 7 1—> / ? 7 defines a morphism of topological monoids,
?/ : QX —»· QY.
In particular, suppose F -? ? -^» ? is a fibration with path connected base,
Y and path connected fibre, F. Choose basepoints yo € Y, xq € X and zq € F
so that F = Xyo and j(.zo) = xo· It follows easily from the exponential law and
Proposition 2.1 that QX -? ?? is a fibration with fibre ?^. Moreover the
multiplication in QX restricts to an action of QF on QX, and so the pair
QX % QY , QXxQF^ QX
(c) The homotopy fibre and the holonomy fibration. Let / : X —> Y
be a continuous map between path connected spaces and let <7o,<7i ·" MY —> Y
denote the maps G, t) 1—> 7@) and G, t) 1—> j(?). Let cy be the path of length
0 at y. Form the commutative diagram
X xY MY · X xy MY is the fibre
product with respect to
, where / and go
• j(x) = (x,cfx)
It is easy to verify that j is a homotopy equivalence and g is a fibration: we say
the diagram converts f into the fibration q.
Fix a basepoint y0 ? Y. The fibre of q at y0 is I x}- PY. It is called the
homotopy fibre of f. Clearly the action of QY on PY defined above defines an
action of QY on the homotopy fibre. Moreover the diagram
PY
, ? = projection on the first factor,
Homotopy Theory
31
exhibits ? as a pull back HF-fibration. It is called the holonomy fibration asso-
ciated with the continuous map f : X —> Y.
Let ? : ? ?? MY -t X be the projection; it is a homotopy equivalence
inverse to j. The commutative diagram
?' xYPY
? ? ? MY
?
?
identifies ? with i and / with q, 'up to homotopy'. Thus the long exact homotopy
sequence for the fibration q translates to a long exact sequence
irn(X ?? PY)
???(?')
???(?)
xY PY)
Finally, write F = f~1(yo)· Then j restricts to an inclusion jo : F —>
X xY PY.
Proposition 2.5
(i) If ? is a fibration then (? ? ? PY,F) is a DR pair. In particular, jo is a
homotopy equivalence.
(ii) If ? is a Serre fibration then jo is a weak homotopy equivalence.
proof: (i) Define a commutative square of continuous maps
X xY PY ? {0}
X xY PY ? [?,??)
X
Y
by setting ?(?, -y, t) = ~f(t). Lift ? to a continuous map ? : ? ?? PY ? [0, oo)
X so that ?? = ? and ?(?,7,0) = ?.
Then a homotopy ? : X xy PY x I —> ? ?? PY is given by
where ?? is the length of 7 and jt is the path of length A - t)?7 given by jt(s) =
?{?? + s). Clearly ii is a homotopy rel F from the identity to a retraction
X xy PY —> F. Finally, if h : X x Y PY —> / is denned by h(x, 7) = inf A, L{)
then /?-1@) = F.
CuJ This follows immediately from Proposition 2.3 since j is a homotopy
equivalence. D
32 2 Fibrations and topological monoids
On the other hand, we can exhibit the homotopy fibre Ff of / : X —> Y
as the analogue of a homogeneous space. Let P'X C MX be the subspace of
Moore paths G,^) that begin at the basepoint xq : 7@) = xo- Multiplication of
paths defines a left action of ?? on P'X and the projection
? : P'X xxFf —»· Ff 2G,2) .—>¦ ?
is a (left) ??-fibration.
Let c_x and cy denote the constant paths of length zero at the basepoints xo
and 2/0 = fxo- Then QX is the actual fibre of ? at the basepoint (??,??) of F/
and the fibre inclusion is given by
? : ?? —> P'X xx Ff, ? : 7 ·—»¦ G * Cx,zo,Cy).
Thus we may regard F/ as the 'quotient' of P'X ? ? Ff by the topological monoid
??.
Now recall that Ff = X xy PF- Thus P'X xx Ff = P'X xx ? ? ? ??.
Define a continuous map
?-.P'XxxFf—^QY byr?G)x)w)
We show that ? has the following properties:
• 77 is a homotopy equivalence.
• 77G · z) = (?/h * ryz, 7 e ??, ? € P'X ?? F/.
• The diagram
?? 3- P'X ?? Ff
commutes.
Thus 'up to homotopy equivalence' the ??-spaces P'X ?? Ff and QY coincide
and so F/ may be thought of as the quotient of ? ? by ??.
Of the three properties listed for ? the last two are immediate consequences
of the definition. For the first, notice that P'X x* X xy PY = P'X xY PY
and that the projection on the first factor is a fibration P'X xy PY —>· P'X,
with fibre ??. Since P'X is contractible the inclusion ? : ?? —> P'X ? y PY of
the fibre is a homotopy equivalence. But ?? = identity and so ? is a homotopy
equivalence too.
(d) Fibre bundles and principal bundles.
A product bundle with fibre ? is a. continuous map ? : X —^ Y for which there
is a homeomorphism g : ? ? ? —? ? such that pg(y, ?) = y. A fibre bundle with
Homotopy Theory 33
fibre ? is a continuous map ? : X —> Y such that Y is covered by open sets
?? and the restrictions pi : p~l(Oi) —> O{ are product bundles with fibre Z. If
? : X —y Y is a fibre bundle and / : A —> Y is any continuous map then the
pullback ? ? ? ? —> A is also a fibre bundle.
Proposition 2.6 A fibre bundle is a Serre fibration.
proof: First note that if a continuous surjection ? : X —» Y has the lift-
ing property with respect to (/" ? /,/" ? {0})?>0, then it is a Serre fibra-
tion. Indeed, suppose given a relative CW complex (W, A) and a homotopy
g : Wxl —»· Y. Note that (Dn ? /.S" x IU Dn ? {0}) S (/« ? /,/« ? {0}).
Thus if / : W x {0} U ? ? ? —> ? satisfies pf = g we may extend /, one cell at
a time, to a lift of g.
Suppose now that ? : X -> Y is a fibre bundle and we want to lift g : In+1 —>
Y through ? extending f : In ? {0} —> X. Use the pullback over g to reduce
to the case Y — 7n+1, g = identity. Recall (§1) that a subdivision of / gives a
product cellular structure for /n+1. Choose the subdivision sufficiently fine that
each 'little cell' is contained in an open set over which the fibre bundle is trivial.
Then the restriction to each cell is a Serre fibration and the construction of the
lift g, one cell at a time, is immediate. ?
Now consider the following situation:
• ? : X —> y is a continuous map.
• Y is a CW complex with characteristic maps Fa : D% —> Yn-
• The pullbacks D% x ?? —> D% (all a, n) are product bundles with common
fibre Z.
Proposition 2.7 With the hypotheses above ? : X —y Y is a fibre bundle with
fibre Z. In particular it is a Serre fibration.
proof: For each fc, the characteristic maps define a homeomorphism
U {d\ - S*) A yfc - yfc_!. Thus the restriction ? : p~l{Yk ~ Yk-i) —>
Yk — Yk-i is a product bundle over the open subset Yk — Yk-i of Yk.
Suppose now by induction that we have extended this to an open set U C
Yn-i and a homeomorphism h\j : U x Z —i p~l{U) such that phu(u,z) — u.
We shall extend U to -an open subset V C Yn and hu to a homeomorphism
hv : V ? ? -=> ? (F) such that phv(v,z) = v.
For simplicity put D = [] D% and 5 = U^ and write Yn - Yn-\ U/ D
a ., a
where / : 5 —>¦ Yn_l is the attaching map. Then identify D% - {oa} = S^ x
@,1], with {oa} the origin of D% and 52 identified with 5J" x {1}. Thus
34 2 Fibrations and topological monoids
D - UM = S ? @,1] and V = U U, (f~l(U) x @,1]) is an open set of Yn
extending U.
Next we undertake the extension of hu to hv- The hypothesis above provides
a homeomorphism ? : D ? ? —l· D ?? X-, compatible with the projections on D.
Moreover hu pulls back to the homeomorphism hf : f~l(U)xZ -^ /~1(??)???
given by hf(w,z) = (w,h(fw,z)). Thus ?~? ? hf is a self homeomorphism of
f~l(U) ? ? of the form A0,2) 1—> (w,ip(w,z)). Extend this to the homeomor-
phism
Of : rl{U) x @,1] ? ? A {rl(U) ? @,1]) xY X
given by 9f(w,t,z) = ? ((w,t),ip(w,z)).
The homeomorphisms /i?/ and ?/ are compatible and so define a unique set
theoretic bijection hv making the following diagram commute:
(U U rl{U)x{Q,l})xZ{I^a{UxYX) ? {{r\U) ? @,1}) ?? ?)
qxid
V ? ? p-\V)
in which q is the obvious quotient map and q' is projection on X. Since hu and
Qf are homeomorphisms we need only verify that q x id and q' are quotient maps
to conclude that hv is a homeomorphism too.
Since g is a quotient map so is the product q ? id. To consider q' write A =
Fif'1^) x @,1])- Then U and A are closed in V and
F = {/ U A. Since <?' = id : U ?? ? -=» ?~?(?/) it is enough to show that
q' ¦ (/-1(i/) x @,1]) ?? X —> p~1(A) is a quotient map. Because we work
with fc-spaces this reduces to the assertion: if ? C Kcomp*ct C p~1(A) and if
(q')~l(B) is closed, then ? is closed.
But the compact set p{K) is covered by finitely cells Fai (?";), 1 < i < N. Put
?
C = \J F~^(pK). Thus C and hence C ?? ? are compact. Since p(K) C A,
il
C C f~l{U) ? @,1]. Thus (g1)^) n (C x^ ^) is compact. A simple check
shows that ? - q' {{q'Y^i?) nC ?? ?). Thus ? is compact, and so closed in
p~1(A). This completes the proof that q' is a quotient map and hence shows
that hy is indeed a homeomorphism.
This inductive construction leads to a subset ? C Y and a bijection /? :
? ? ? —>· ?'1 (?) with the following properties:
for ? > fc, On ¦= ? ? yn is open in Yn.
^ " ^ " ™~1(O..) is a homeomorphism.
Homotopy Theory 35
Thus ? is open in Y. Moreover, since any compact subspace of Y is contained
in some Yn (Proposition 1.1 (ii)) it follows that C C ? ? ? is compact if and only
if h(C) is compact and that h : C -=> h(C)· This implies (because we work in
the category of fc-spaces) that h is a homeomorphism. ?
We turn now to the definition of topological groups and principal (fibre) bun-
dles, which are important special cases of topological monoids G and G-Serre
fibrations.
A topological group is a topological monoid G such that the monoid is a group
and the map g \—> g~l is continuous. If the topological group G acts continu-
ously on a topological space X then X is the disjoint union of the orbits x-G,
and the orbit space X/G is the set of orbits equipped with the quotient topology.
(We only consider cases where X/G is Hausdorff.)
Let G be a topological group. A principal G-bundle is a continuous map
? : X —> Y together with an action of G on X such that Y is covered by
open sets Oa and there are continuous maps ?? : Oa —> X with the following
properties:
• ??? = id
• A homeomorphism Oa ? G —i p~l(Oa) is given by (y,g) ?—> cra(y) · 9-
In this case ? : X —» Y is a fibre bundle with typical fibre G, the orbits of G
are the fibres of ? and ? induces a homeomorphism X/G ^> Y. In particular,
? : X —> Y is a G-Serre fibration.
Now consider the following situation:
• ? : X —y Y is a continuous map and A' x G —> X is a continuous action
of a topological group G.
• Y is a CW complex with characteristic maps Fa : D? —> Yn.
• There are continuous maps ?? : D% —> D% xy X (all ?,?) such that ??
has the form ??(??) = (?),??(??)) and
is a homeomorphism.
Proposition 2.8 . With the hypotheses above ? : X —> Y is a principal G-
bundle.
Proof: It follows from Proposition 2.7 that ? : X —>¦ Y is a fibre bundle.
Thus Y is covered by open sets Ui for which there are continuous maps r,· :
^ *¦ X such that ??{ = id. We have only to check that the continuous maps
"-i :Ui ? G —> P~l{U{), (u, g) ?—> Ti(u) ¦ g, are homeomorphisms.
36 2 Fibrations and topological monoids
It is immediate from the hypotheses above that hi is a bijection. Thus
it is sufficient to show that /^'(C) is compact for any compact subspace
C C p~l(Ui). Since p(C) is covered by finitely many cells (Proposition l.l(ii))
this too follows easily from the hypotheses. ?
(e) Associated bundles, classifying spaces, the Borel construction and
the holonomy fibration.
Suppose given a principal G-bundle ? : X —> Y and an action of G on a third
topological space Z. Then G acts diagonally on ? ? ? : (?,?) · g = (xg,zg).
Consider the continuous maps
? ??? (X xZ)/G^Y
where q is the map of orbit spaces induced by the projection ? ? ? —> X. It
is an easy exercise to check that ? is the projection of a principal G-bundle and
that q is the projection of a fibre bundle with typical fibre Z: this fibre bundle
is called the fibre bundle associated to the principal bundle via the action of G
on Z.
Central to the study of principal G-bundles is Milnor's universal G-bundle
[125]
pG:EG^ BG
constructed as follows.
Recall (§l(f)) that CG = (G ? I)/G ? {0} is the cone on G, and that the nth
join, G*n, is the subspace of CG ? ¦ ¦ ¦ x CG of points ((g0, ??), · · ·, (9n, tn)) such
that ??? = 1. Thus G*n C G*(n+1) (inclusion opposite the base point of CG).
Set EG = (J G*n, equipped with the weak topology determined by the G*n.
?
A continuous action of G in EG is given by
((go,to),...,(gn,tn)) ¦ g = ((gog,t0),¦¦-,(gng,tn)).
Set BG = EG/G and let pG : EG —> BG be the quotient map. It is an easy
exercise to verify that
• pG : Eg —> BG is a principal G-bundle.
• every continuous map from a compact space to Eg is homotopic to a
constant map. In particular, nt(EG) = 0.
The space BG is called the classifying space of G. Now associated with an
•arbitrary action of G on a topological space X are two important constructions:
the Borel construction and the loop construction.
The Borel construction is the fibre bundle
with typical fibre X, associated to the universal bundle via the action of G on
Homotopy Theory
37
Proposition 2.9 If ? : X
homotopy equivalence Xg —
Y is a principal G-bundle then there is a weak
Y.
proof: The associated fibre bundle with fibre Eq has the form
q' : XG = (EG x X) IG -i- Y.
Since ?*(??) = 0 and since this is a Serre fibration, the long exact homotopy
sequence shows that q' is a weak homotopy equivalence. ?
Consider a principal G-bundle ? : X —> Y whose base y is a CW complex.
Because q' : Xg —> Y is a weak homotopy equivalence there is a map ?' :
Y —> Xg such that q'a' <~ id (Whitehead Lifting Lemma 1.4). Because q' is
a Serre fibration we can lift the homotopy starting at a' to obtain a homotopy
?' ~ a : Y —> Xg with q'a = id. This identifies Y as a subspace of Xq-
Restrict the principal G-bundle Eq xX —> Xg to a principal bundle ? —> Y.
Projection Eg ? X —> X restricts to a map ? —> X of principal bundles covering
the identity map of Y. This map is therefore a homeomorphism, which we use
to identify ? = X.
Thus the diagram
X
Y
EGxX
Eg
Bg
exhibits the original principal bundle as a pullback of Milnor's universal bundle
(whence the name). An extension of this argument shows that the pullback
construction defines a bijection
isomorphism classes of principal
G-bundles over Y
thereby explaining the terminology 'classifying space' for Bg-
Finally, suppose a topological monoid G acts on a path connected space X with
basepoint x0. Then, as described in §2(c), the continuous map a : G —> X, a :
9 ?—> xo ¦ p, can be converted into the fibration
G ?? ? ? —>¦ G ? ? MX —> ?. Moreover, this is homotopy equivalent to
GxxPX
X
where ? is the projection of an ??-fibration.
Now we show that G ? ? ?X is a topological monoid and that ? is a morphism
of topological monoids. Thus X may be thought of as a sort of 'generalized
38 2 Fibrations and topological monoids
homogeneous space'. To construct the multiplication on G xj PX let G act on
MX by (w ¦ g)(t) = w(t) -g,w 6 MX, g eG. Then set
(9, w) ¦ (g',w') = {gg, (w ¦ g') * w'),
where, as usual, * denotes composition of paths. The pair (ec, cXo) is a two sided
identity. We call the monoid G ? .? PX the loop construction at x0 corresponding
to the action of G on X.
In particular, suppose given an arbitrary principal G fibre bundle
? -^ ? , ZxG -^ ?
in which ?»(?) = 0. Then morphisms of topological monoids
are defined by 7E, u;) = g and 7'(<7, to) = ps ? «j .
Proposition 2.10 7 anei 7' are weak homotopy equivalences, so that G and
VtB are weakly equivalent topological monoids.
proof: Define an action of G ? ? PZ on PZ by setting u-(g.w) =
(u-g) * w. Then ? : PZ —>¦ B, n(w) = pB(u>@)), is a G xz PZ-fibration.
It fits in the diagram of fibrations
? +-1— PZ —^ PB
where f(w) = w@) and f'(w) — pB out. Moreover / and /' restrict to 7 and 7'
respectively in the fibres. Since ?» vanishes on ?, ? ? and PB, it follows from the
long exact homotopy sequence that 7 and 7' are weak homotopy equivalences.?
Observe that in the proof of Proposition 2.10 that G,7) and (f',j') are equiv-
ariant weak equivalences.
Again let ? : ? —> ? be the principal G-bundle described just before Propo-
sition 2.10. If ? : Y —> ? is any continuous map we can form the pullback
principal bundle
? : ? ?? ? -^ ? , (? ?? ?) ? G -^ Y ?? ?
and also the holonomy fibration (§2(c)),
n:Yx.nPB—>Y , (Y xB PB) ? UB -^ Y xB PB .
Homotopy Theory 39
The diagram of Proposition 2.10 pulls back to the diagram of Serre fibrations
? Xe ? . YxBf Y x? PZ YXBf' - Y x? PB
Y
which identifies ? ? ? / and ? ? ? /' as equivariant weak equivalences. This
extends Proposition 2.10 to
Proposition 2.11 The pullback fibre bundle ? : ? ?? ? —? ? and the holon-
omy fibration of ? are connected by equivariant weak equivalences of fibrations:
Y xBZ
Y xB PB
G
Exercises
1. Let F be the homotopy fiber of the inclusion i : X VY -? X xY. Prove that
F ~ ?.? * ?.? and that Qi is a QF- fibration with a cross section. Assume that
A' and Y are simply connected CW complexes. Prove that Q(X V Y) is weakly
homotopy equivalent to the product ?.? ? ?1' ? UF. Deduce that if Ar (resp.
Y) is r (resp. s) connected then nk(X V Y) = irk(X) © 7rfc(y) for k < r + s — 2.
2. Let X be a locally compact space and .4 c—> X a closed cofibration. Prove
that the canonical map Yx -t YA is a fibration.
3. Prove that ? : ?1 -> ? ? ? = ?91, f ?- (/(?), /(I)) is a fibration. Prove that
q : Bs -? ?, q(j) = 7@) is the pull-back fibration of ? : ?1 -> ? ? ? along the
diagonal map ? -> ? ? ?. Deduce that nk(Bs') = nk(B) ? 7rfc+1 E), k > 1.
4. Let / : Sn -? Sn be a homeomorphism of degree —1 and ? the quotient
space of the product Sn ? [0,1] by the relation (a-,0) ~ (f(x), 1). Prove that the
composite Sn ? [0,1] -> [0,1] -> S1 induces a fibration ? : ? ^ S^. For ? > 2,
compute 7rfe(?) and the action of US1 on the homotopy fibre Fp ~ Sn.
5. Prove that if X and y are simply connected CW complexes then the homotopy
fibre of the pinch map X V Y -> X is the half-smash product ? ? ?.?/ * ? ??.
3 Graded (differential) algebra
In this section we work over an arbitrary commutative ground ring k, except
in (e). Thus module, linear, bilinear, ... will mean k-module, k-linear, k-
bilinear, ... and the functors ????^(—, — ) and — ®jj; — will be denoted simply
by Hom( —, —) and ~ <g> —.
The object of this section is to establish the basic definitions and conventions
of graded algebra upon which the rest of this book will rely. It is organized into
the topics:
(a) Graded modules and complexes.
(b) Graded algebras.
(c) Differential graded algebras.
(d) Graded coalgebras.
(e) When k is a field.
(a) Graded modules and complexes.
A graded module Vy is a family {Vi};6z of modules. By 'abuse of language'
we say that an element ? 6 V{ is an element of V of degree i, and we write
|u| — degu = i. We say that V is concentrated in degrees i 6 / if Vj· = 0,
i ? I , and, by abuse of notation, we write V = {Vi}ieI. In particular we write
Veven = {V2i}iez and Fodd = {V2i+1 }iez-
Notice that we make frequent use of the Koszul sign convention that when
two symbols of degrees k and i are permuted the result is multiplied by (—l)ke.
The standard notions for non-graded modules carry over to the graded context:
• A submodule V C V is a graded module {V/} with V{ C V;.
• The quotient V/V of a module by a submodule is the family {Vi/V-}.
• The direct sum ©aV(a) is the family {®aV(a)i}. In particular if V(i) is
the graded module defined by
0 , otherwise
then V = ? V(i). We shall therefore sometimes abuse notation and write
The direct product f] V(a) is the family {]7 V(a)i}. The direct product of
a a
V and W is written V ? W.
Homotopy Theory 41
• A graded module, V, is free if each Vi is a free module. In this case the
disjoint union of bases of the Fj is called a basis for V.
• A linear map f : V —> W of degree i (between graded modules) is a
family of linear maps fj : Vj —> Wj+i- It determines graded submodules
ker/ C V and Im/ C W : (ker/),· = ker/,· and (Im/)j = Im/j_i. We
denote by Hom(V, VI7) the graded module whose elements of degree i are
the linear maps of degree i. If / : V —> V and g : W —> W are linear
maps then Hom(/,<?) : Horn(F, IF) —>¦ Hom(V,W) is the linear map
? ? >· (_l)deg/)^
• The module Hom(F, Je) dual to F will be denoted by T/jt, and fi =
Hom(/, Jfc) : W" —>¦ Fc will denote the dual of the linear map /.
• Suppose fa : V(a) —> ? are linear maps of degree zero. The fibre product,
( ? )z(V(a)), is the submodule of f] V(Q) consisting of the elements
a61 a6l
{va} satisfying fa(va) — f?(v?), ?, ? El. The fibre product of V and W
is written V ? ? W.
• The tensor product V <g> W of graded modules is defined by (V ® H7)! =
$y+fc=i Fy ® VFfc. If / : V —> V and 0 : W —>¦ VF' are linear maps of
degrees ? and g, then f ® g :V ®W —> V <8> W is the linear map:
(/ ® </)(« ® w) = (-l)de" de8"/(«) ® ?/(?)
of degree ? + q. If / : F —> V we frequently (but not always) simplify
/ ® idw to/®W:V®W-+V® W.
• A p-linear map is a map ? : V"(l) ? ··· ? V(p) —> W of the form
a(vi ,...,Vp) = ?(vi ® · · · ® vp), where ? : V\ ® · · · ® Fp —^ IF is a
linear map. A bilinear map V ? W —>¦ Jfc is called a pairing and is often
written u, u; ?—>· {?,?). It determines (and is determined by) the linear
map ? : V —? W^ given by {??)?? = {v,u>). In particular, if it is a field
and F" and IF" are the dual graded vector spaces, then Fo ® W* is the
subspace of (V ® IF1K given by
(v'®w',v®w) = (-l)de&w'de&v(v',v)(w'1w).
In addition,
• The suspension of a graded module V is the graded module sV defined by
(sV)i = Vi-i. If ? e ?,_! the corresponding element in (sVr), is denoted
by sv.
• We shall use the classical convention
42 3 Graded (differential) algebra
to avoid negative degrees. Thus the degree of an element will depend on
the context, depending on whether we are using upper or lower degrees.
For example, if / : V —> W has degree -1 with respect to lower degrees,
(/ : V;· —>¦ Wj-i), then it has degree +1 (/ : V* —>¦ W/i+1) with respect
to upper degrees. In this section 'degree' will always mean 'lower degree'
unless otherwise specified.
• We use the notation V>k = {Vi}i>k and V>k = {Vi}i>k- The graded sub-
modules V<k, V<k, V>k, F-*, V<k, V^k are defined analogously. More-
over we write
V+ = {?%>0 and V+ = {Vi}i>0 .
A sequence M —> ? -^ Q of linear maps is exact if kerg = Im/. If also /
is injective and g is surjective then 0 —? M —? ? —> Q —? 0 is a short exact
sequence. A routine check establishes
Lemma 3.1 (Five lemma) Suppose given a commutative row-exact diagram of
linear maps of graded modules
(i) If ctL is surjective and a m and &P are injective then ?/? is injective.
(ii) If auf and ap are surjective and q.q is injective then a^ is surjective.
(Hi) If OiL is surjective, ac is injective and am and cap are isomorphisms then
? ? is an isomorphism. D
A differential in a graded module M = {A/;}igz is a linear map d : M —? ?
of degree -1 such that d2 = 0, and the pair (M,d) is called a complex. The
elements of ker d are cycles, the elements of Im d are boundaries and the quotient
graded module H(M,d) = kerd/lmd is the homology of M. We often simplify
the notation to H{M).
A morphism of complexes ? : (M, d) —> (?, d) is a linear map ? : M —y N
of degree zero satisfying ?? = ??. It induces ? (?) : H (M) —> H (?). If H (?)
is an isomorphism, ? is called a quasi-isomorphism and we write ? : M -^4 ??.
Two morphisms ? and ? are homotopic if there is a linear map h : M —>¦ ?? of
degree 1 such that ?~? = hd + dh. We write ? ~ ? : {M,d) —>¦ (iV,d) and call
h a chain homotopy.
A chain equivalence ? : (M, d) —>¦ (?, d) is a morphism such that there is
a second morphism ? : (N,d) —> (M,d) satisfying ?? ~ id^ and ?? ~ idm-
Since ? {?) and ? (?) are then inverse isomorphisms, a chain equivalence is a
miasi-isomorphism.
Homotopy Theory 43
If (M, d) and (TV, d) are complexes, then so are Hom(jl/, N) and ? ® ??:
d(f) = df - (-l)des//d, / 6 Hom(il/, N), and
d(m ®n) = dm®n + (- l)des mm®dn, me ?, ? 6 ?.
Note that in particular the differential in Hom(il/, lz) is the negative of the dual
of the differential in M.
Suppose / is a cycle in Hom(M, iV); i.e. df = (-l)de&ffd. Consistent with
the notation above we define a linear map H(f) : H(M) ->· H(N) by H(f)[z] —
[f(z)], where [ ] denotes 'homology class'.
Lemma 3.2 ^4 necessary and sufficient condition for H(f) to be an isomor-
phism (of degree i) is that for each (??,?) ? ? ? ? satisfying dm = 0 and
/(m) = dn there exist {m',n') 6 ?/xiV satisfying dm' = m and dn' — n — f(m').
proof: Suppose the condition holds. If dm = 0 and H(f)[m] = 0 then /(m) =
dn; hence m = dm' and [m] = 0. If [n] 6 H(N) then dn = 0 = /@) and so there
exist (m'.n') with dm' = m and dn' — ? - f(m'); i.e. [n] = H(f)[m'].
Suppose H(f) is an isomorphism. Given (m,n) as in the lemma we have
H(f)[™] = 0· Hence m = dm". Now ? - f(m") is cycle; hence [n - f(m")] =
H(f)[z] = [/(«)]. Thus m = d(m"+z) and n-/(m" + 2) = tin'. Set m' = m"+z.
D
A chain complex is a complex (M,d) with A/ = {?/?}?>?; a cochain complex
is a complex (M,d) with ?? = {?·/?}?>0. In the latter case d has (upper) degree
1, the elements of kerei are cocycles, the elements of Im ci are coboundaries and
H(M, d) is the cohomology.
The suspension of a complex (il/, d) is the complex ?(?/, d) — (sM, d) defined
by sdx = —dsx.
A short exact sequence of morphisms of complexes, 0 —> (il/, d) —i (N, d) —>¦
(Q, ei) —^ 0 induces a Zong exaci homology sequence,
, H.{M) W> Hl(N) -^ Hi(Q) ? tfi-i(M) ->·.·,
defined for all i, in which the connecting homomorphism d is defined in the usual
way: if ? 6 Q represents [z] 6 Hi(Q) and if /3n = ? then 3[s] is represented by
the unique cycle ? 6 M such that ax = dn.
(b) Graded algebras.
A graded algebra is a graded module R together with an associative linear map
of degree zero, R®R —> R, x®y ?—> xy, that has an identity 1 6 Ro. Thus for
^1 x,y-, ? 6 R (xy)z = x{yz) and \x = ? = xl. We regard k as a graded algebra
concentrated in degree 0. A morphism ? : R —> S of graded algebras is a linear
map of degree zero such that <+>{xy) = ?(?)?(?) and <p(l) = 1.
44 3 Graded (differential) algebra
An augmentation for a graded algebra R is a morphism ? : R —> L· oi graded
algebras.
A derivation of degree it is a linear map ? : R —> R of a degree & such that
e{xy) = {9x)y + {-l)ka*zxx{9y).
Let fibea graded algebra. A (left) R-module is a graded module M together
with a linear map of degree zero R® M —> ?, ? ® m ?—> im, such that
x(ym) = (xy)m and Im — m for all ?, y G i? and m 6 M. Right modules are
defined analogously.
An R-linear map f : M —> ? of degree k is a linear map of degree k such
that
/(im) = (-l)deg/ degIx/(m), xeRy me M.
These form a graded submodule ?????(?, ?) of the graded module
Hom(M,iV).
The tensor product M' ®n M of a right A-module M' and a left A-module M
is the quotient module
M' ®n M - (M' ® M)/(m'x ®n-m' ® xn),
where we have divided by the submodule spanned by elements of the form m'x ®
? - m' ® xn, m' 6 M', m ? ?, ? 6 R. We use m' ® m to denote the obvious
element in M' <g> M\ its image in M' ®r M, is denoted by m' ®r m.
Let R be a graded algebra. A left ideal I in R is a graded submodule such
that xy € I for ? 6 R and y ? I. Right ideals, (two sided) ideals and subalgebras
are defined analogously. Note that subalgebras must contain 1. The quotient
R/I of R by an ideal / is a graded algebra.
Example 1 Change of algebra.
A morphism ? : R —> S of graded algebras makes 5 into a left (and right)
A-module
? · s = tp(x)s or s · ? = s<p(x), ? € R, s 6 5.
If M is an .R-module then S ®h M is an 5-module via s · (s' ®r m) = ss' ®r m.
D
Example 2 Free modules.
Let R be a graded algebra. An A-module M is free if M S R ® V, with V a
free graded module. A basis {va} for F is a basis for the free A-module M. If
? : R —> 5 is a morphism of graded algebras then
5 ®n M = S ®R (R <g> V) = S ® Vr
is a free S-module with the same basis.
If M is a free A-module with basis {va} and if iV is any i?-module, then the
choice of elements na 6 Nk+^^ determines a unique A-linear map / : M —> ?
of degree k with f(va) = na. ?
Example 3 Tensor product of graded algebras.
Homotopy Theory 45
If R. S are graded algebras then R ® S denotes the graded algebra with mul-
tiplication
(x ® y)(x' <8> y') = (-l)de" d^x'xx' ? yy'.
Example 4 Tensor algebra.
For any free graded module V, the tensor algebra TV is defined by
oc
TV = 0 Tqv Tqv = y ®···®?.
Multiplication is given by ? · ? = ? <g> b. Note that q is no? the degree: elements
v\ ® · · · ® vq 6 TqV have degree = ? degu; and word length q. If {uj} is a basis
of V we may write TV = ?{{?{}).
Any linear map of degree zero from V to a graded algebra R extends to a
unique morphism of graded algebras, TV —>¦ R. Any degree k linear map
y —>¦ TV extends to a unique derivation of TV. ?
Example 5 Commutative graded algebras.
A graded algebra /I is commutative if
x,y ? A .
When i 6 1 this condition implies that x2 = 0 if .? has odd degree. If A is a
commutative graded algebra, then a left yl-module, M, is automatically a right
.4-module, via
mx = (-l)desmdesxzm .
If ? is a second ?-module then Horn a (M, iV) and ?/ «gi^ iV are .4-modules via
(x/)(m) = ? ¦ /(m) = (-l)desx dee//(xm)
and
x(m ®? ?) = xm <8u ? = (-i)desx deg771m ®.4 in, ? e A, m 6 ?, ? 6 ? .
If -4 —> ?, .4 —^ C are morphisms of commutative graded algebras then
? ® C is also commutative and the kernel of the surjection ? ® C —> ? <8u C
is an ideal. Thus ? ®A C is also a commutative graded algebra. D
Example 6 Free commutative graded algebras.
Suppose Jk contains |. Let V be a free graded module. The elements v®w —
(_l)deg« dezwWQV (v^w 6 y·} generate an ideal / C TV. The quotient graded
algebra
AV = TV/I
is called the free commutative graded algebra on V. If {iij} is a basis of V we
may write AV = ?({?,}). The algebra AV has the following properties:
46 3 Graded (differential) algebra
(i) ? I·'' is graded commutative; in particular, the square of an element of odd
degree in AV is zero.
(ii) There is a unique isomorphism A(V®W) = AV<S>AW which is the identity
in V and in W.
(iii) If V is free on a single basis element {v} then a basis of AV is given by:
1 ? if deg ? is odd
1 ? ?2 ?3 if deg ? is even.
(iv) A linear map of degree zero from y to a commutative graded algebra A
extends to a unique morphism of graded algebras, Ay —> A. A linear map
y —> Ay of degree k extends to a unique derivation in Ay.
(v) Suppose ? : AV -4 A is a morphism of graded algebras and ? and ?' are
derivations respectively in Ay and in A. If ??? = ?'??, ? 6 V, then
?? = ?'?.
??
(vi) Ay = ? ?9 y, where ?9 y is the linear span of the elements v\ ?···??M,
9=0
Vi & V] these elements have degree = ?* deg v, and word length q.
(c) Differential graded algebras.
A differential graded algebra (dga for short) is a graded algebra R together with
a differential in R that is a derivation. In this situation kerd is a subalgebra and
Imd is an ideal in kerd. The homology algebra H(R,d) of a differential graded
algebra (R.,d) is the graded algebra H(R,d) = kerd/Imd. An augmentation is
a morphism ? : (R, d) -4 it.
A morphism of differential graded algebras / : (R, d) —> E, d) is a morphism
of graded algebras satisfying fd = df. It induces a morphism H(f) : H(R) —>
H(S) of graded algebras.
If (R, d) and E, d) are dga's then so is (R, d) ? E, d) with the differential
described in (a) and the multiplication given in Example 3 of (b). This is called
the tensor product dga.
A chain algebra is a dga {R,d) with R = {Rn}n>0. A cochain algebra is a dga
(R, d) with R = {Rn}n>o- In the latter case H(R,d) is the cohomology algebra
of (R,d).
A (left) module over a differential graded algebra (R, d) is an A-module, ?/,
together with a differential d in M satisfying
d(x ¦ m) = dx ¦ m + (-l)degrx · dm, ? ? R,m ? M.
Homotopy Theory 47
Then H(M) is an H(R)-modu\e via
[x] ¦ [m] = [x · m].
A morphism of (left) modules over a dga (R,d), is a morphism
/ : (M, d) —i (N, d) of graded i2-modules satisfying df = fd. The induced
map H(f) : H(M) —> H(N) is then a morphism of H(R)-modu\es.
If (M, d) and (JV, d) are left (R, demodules, then Hom/j(M, N) is a subcomplex
of Hom(M, ?1'). In the same way, if (M'.d) is a right (R, d)-module then the
differential d defined on M' <g> AI induces a differential in M' ®r M so that
M' ®r M is naturally a complex.
Example 1 Hom#(M, M).
If (M,d) is any (R, d)-module, then Hom^(M,M) is a differential graded
algebra with multiplication defined by the composition of maps. ?
Example 2 Tensor products.
If (A,d) -> E, d) and (A,d) -> (C,d) are morphisms of commutative dga's
then E ®A C,d) is a commutative dga. D
Example 3 Direct products.
If (A, d) and (A',d) are dga's then the direct product (A, d) ? (.4', d) is the dga
(A x ^4',d) given by (a,a') · (??,??) = (???,?'??) and d(a,a') = (da,da'). The
direct product, Y\a(A(a),d), of a family of dga's is defined in the same way. ?
Example 4 Fibre products.
If / : (A,d) -> E,d) and /' : (A',d) -> E,d) are dga morphisms then
(A xb A',d) is a sub dga of (.4, d) x (A',d). It is called the fibre product of
(^4,d) and (^4',d). The fibre product of a family of dga's (^4(a),d) with respect
to dga morphisms fa : (A(a),d) -> (B,d) is the sub dga ((Y\a)BA(a),d) of
ne(^("),d)· D
(d) Graded coalgebras.
A graded coalgebra C is a graded module C together with two linear maps of
degree zero: a comultiplication ? : C —> C®C and an augmentation ?: C —>¦ h
suchthat (A®id)A = (id®A)A and (id<S>e)A = (e®id)A = idc- A morphism
? : C —^ C of graded coalgebras is a linear map of degree zero such that
(<p® ?) ? = ?'? and ? = ?'?. A graded (left) comodule over a graded coalgebra
C is a graded vector space M together with a linear map Am : C —> C ® M of
degree zero such that (? ® id) Am = (id<8> Am) Am and (e ® idM)C = id^.
A graded coalgebra is co-commutative if
?? = ?
where r : C <g> C —>¦ C <g> C is the involution ? <g> 6 ?—> (-i)deea dee66 ® a.
48 3 Graded (differential) algebra
A graded coalgebra is coaugmented by the choice of an element 1 € Co such
that e(l) = 1 and ?A) = 1®1. Given such a choice the relations above imply
that for ? € kere,
?? - (? ® 1 + 1 ® a) € ker ? ® ker ? .
An element, a, in a coaugmented graded coalgebra is called primitive if ? 6
ker c and ?? = ?<8>1+1®?. Primitive elements form a graded submodule of ker ?,
and a morphism of coaugmented graded coalgebras sends primitive elements to
primitive elements.
A coderivation of degree k in a graded coalgebra C is a linear map ? : C —> C
of degree k such that ?0 = (? ® id + id ® 0)? and ?? - 0.
A differential graded coalgebra (dgc for short) is a graded coalgebra C together
with a differential that is a coderivation in C.
If C is a graded coalgebra, then Hom(C, Ik) is a graded algebra whose multi-
plication is defined by
(f-g)(c) = (f®g)(Ac), f,geHom(C,k), c6C
and with identity the map ? : C —> h. If (C, d) is a differential graded coalgebra,
then C* = Hom(C, Jfc) is a differential graded algebra.
Remark Henceforth we may occasionally suppress the differential from the no-
tation, writing M, R,... for a differential graded module (Ai, d), a dga (R, d),...
(e) When Iz is a field.
Recall that complexes (M,d) and (N,d) determine the complexes ?®?? and
Hom(A/,JV) with d(m <g> n) = dm <g> ? + (-l)desmm ® dn and df = do f -
(_l)deg/yoc^ Natural linear maps
H(M)®H(N)-+H(M®N) and H (Rom(M,N)) —»¦ Horn (?(??),?(?))
are given by [z] ® [w] ·—> [z ® w] and [/] ·—> H(f). (Recall that H(f)[z] =
[Hz)])·
Proposition 3.3. If ]k is a ?e/d these natural maps are isomorphisms:
H(M) ® if(iV) = if(M ® N) and ? (Eom(M, TV)) = Horn (H(M), H(N)).
proof: This is a straightforward exercise using the fact that any complex M
can be written M = Im d © H @ C with d : C -=> Im d and d - 0 in H. ?
In particular, suppose (C,d) is a dgc over a field Jk. Using the first iso-
morphism of Proposition 3.3 we may write ?(?) : H(C) -> H(C) ® H(C).
Together with ?(?) this makes H(C) into a graded coalgebra. The dual algebra,
Homotopy Theory 49
Hom(J?(C), Jk) is just the homology algebra of the dga, Hom(C, k), as follows
again from Proposition 3.3.
If k is a field we say a graded vector space M = {Mi} has finite type if each
Mi is finite dimensional. If also ?/ is concentrated in finitely many degrees then
M is finite dimensional and dim M = ? dim M,.
i
When M has finite type its Hubert series is the formal series
However if M = {M'} we write M*(z) = ?](dimMl)zl. When the context is
i
clear we simply write M(z).
If M is finite dimensional then its Euler-Poincare characteristic is the integer
Xm defined by
?? = ?(-1I dim M, = dim A'Ieven - dimModd = A/*(-l) .
If M is equipped with a differential then it is an easy exercise to verify that
Xm = Xh(m) · C-4)
A graded algebra R (or a dga) has finite type if each Ri is finite dimensional;
i.e. if it has finite type as a graded vector space. Similarly a graded R-module
of finite type is an A-module M such that each Mi is finite dimensional. By
contrast R is finitely generated if it is generated by a finite set of elements (i.e.
if there is a surjection ?(??5... ,Vk) —> R). Similarly M is a finitely generated
e
R module if every m ? ? can be expressed as m = ^Z Xirrii, Xi 6 R, where
mi,... me is a fixed set of elements of M.
Exercises
1. A complex M is acyclic if Hi(M) = 0, i = 0.1,... . Prove that if M is
an acyclic A-free chain complex then icLm ~ 0. Let M and ? be graded chain
complexes. Under what conditions is the complex Horn (M, N) acyclic?
2. Let (?,???) and (N,dN) be chain complexes and / 6 Hom°(A/,N). Prove
that if M is an i2-free module then / is a chain equivalence if and only if the chain
complex (C,d) defined by Ck = Mk-\ ® Nk, d(m,n) = (-dMm,f(m) +dNn) is
acyclic.
3. Consider the free commutative differential graded algebra (A(x, y, z),d) with
deg ? = deg y = deg ? = 1 and dx = yz ,dy = zx ,dz = xy . Prove that the map
? '- (??,?) -> (A(x,y,z).d) defined by ?(?) — xyz is a quasi-isomorphism.
50 3 Graded (differential) algebra
4. Let TV be the tensor algebra on the graded vector space V.
Define ? : TV —»¦ TV ® TV , ? : TV —»¦ A, by
?(?? ® vo <8>... ® un) = 53"=0(?? ® ?2 ® ... ® ^) ® (ui+i <8> vi+2 ® ... ® vn)
e(l) = 1 and ?(?? <S> vo <S> ¦¦¦ <S> vn) = 0 ?? ? > I .
Prove that (T(V), ?, c) is a non cocommutative graded coalgebra.
5. Let (M,<1m) and (N,d^) be chain complexes. Prove that if M is an Et-
iree chain complex then the complex Hom (??. ??) is isomorphic to the complex
Hom {M, R) ® JV.
6. Let (M,cIm) be a chain complex. Prove that if M is of finite type then there
exists a Hubert series Q(z) such that M(z) = H(M)(z) + A + z)Q(z).
4 Singular chains, homology and Eilenberg-
MacLane spaces
As usual, we work over an arbitrary commutative ground ring h.
This section is a quick review of singular homolog}·. Distinct from the usual
approach, however, is our use of normalized singular chains, so as to arrange
that the chains on a point vanish in positive degrees. We also take great care
with the comparison between C,(X xl') and C*(X) eg C*(Y). using the clas-
sical Alexander-Whitney and Eilenberg-Zilber equivalences because these have
important associativity and compatibility properties on which we will subse-
quently need to rely.
Beyond the standard material of elementary singular homology we:
• show that a weak homotopy equivalence induces an isomorphism in homol-
ogy-
• construct the cellular chain complex of a CW complex and show that it is
chain equivalent to the singular chain complex.
• prove the Hurewicz theorem identifying the first non-vanishing homotopy
and homology groups of a space (except when ?\ ? 0, in which case
Finally, we construct the Eilenberg-MacLane spaces ?(?,?) for a group ??
(abelian if ? > 2) and show that if ?' is an (n — l)-connected CW complex then
[?,?(?,?)] = Horn (??(.?), ?), the isomorphism being given by [/] ?—> ???(/).
In fact this theorem generalizes to the famous
Theorem ([E]) For any CW complex X a bisection [?, ?(?, ?)] -=4 ??(?: ?)
is given by [f] ?—> Hn(f)t,, ? denoting the fundamental class of Hn (?(?.?):?).
However we shall not need this result, and so it is not included in the text.
This section is organized into the following topics:
(a) Basic definitions, (normalized) singular chains.
(b) Topological products, tensor products and the dgc. Ct(X;L·).
(c) Pairs, excision, homotopy and the Hurewicz homomorphism.
(d) Weak homotopy equivalences.
(e) Cellular homology and the Hurewicz theorem.
(f) Eilenberg-MacLane spaces
52 4 Singular chains, homology and Eilenberg-MacLane spaces
(a) Basic definitions, (normalized) singular chains.
Recall that a singular n-simplex in a space X is a continuous map
? : ?? —> ? ,
(? ?
where ? > 0 azid ?? = \ ? t{e{ \ 0 < U < 1, ? it = 1 ? is the convex hull of
? ? J
the standard basis e0, - - -, en of En+1. If X C Em is a convex subset then any
sequence xc,, · · -1 ?? € X determines the linear simplex
{x0 ... xn) ¦ An —> ?, ????? h-> ?????.
Two important examples of linear simplices are the i-th face inclusion of ??,
A,· = (eo---ei---en) : ??-1 —> ??, ("means omit)
defined for ? > 1 and 0 < i < n; and the j-th degeneracy of ??,
defined for ? > 0 and 0 < j < ?. The image of Ai is called the i-th face of ??.
Denote by Sn(X) the set of singular n-simplices on a space X (n > 0), and by
Sn(<p) : Sn(X) r Sn(Y), Sn(<p) : ? h-+ ? ? ? ,
the set map induced from a continuous map ? : ? —» ?. The set maps
di : Sn+i(X) -> Sn(X), ? ?-)· ? ? A; and Sj : Sn(X) -> Sn+i(X), ? ?-> ? ? qj
are called the face and degeneracy maps; they commute with the set maps 5»(y).
A straightforward calculation shows that
SiSj = Sj+iSi, , i <j;
( Sj^di ,i<j D-1)
djSj = < id , i = j,j + 1
I Sjdi-i , i > 7 + 1.
The free it-module with basis Sn(X) will be denoted CSn(X; Ik), and the sin-
gular chain complex ?? X is the chain complex CS,(X;Jfc) = {CSn(X;Jk)}n>0,
with differential d — ?(—1-)???. Its homology, denoted H,(X;Jk), is the singular
i
homology of X.
An ? + 1 simplex of the form Sir (r 6 Sn(X), 0 < i < n) is called degenerate
and the degenerate simplices span a submodule DSn+i(X) of C5n+i(„Y;Jfc).
It follows easily (cf. [118]) from D.1) that DS,(X) is a subchain complex of
CS*{X;k) and that H(DS,(X)) = 0. We will use the quotient chain complex:
C»(X; Bt) = CS*(X', Jk)/ DS,(X)
it is called the normalized singular chain complex of X.
The following properties follow immediately from the definitions and the re-
marks above:
Homotopy Theory 53
• Cn(X]fc) is a free module with the non-degenerate n-simplices as basis.
• The surjection CS*(X;]k) —>¦ C.(X;Jc) is a quasi-isomorphism. Thus we
may (and do) use this to identify
H.(X;li) = H (C.(X;lc)). D.2)
• C,(pt) — h, concentrated in degree zero.
(The last property follows because ?? —> pt is degenerate for ? > 1.)
A continuous map / : X —> Y induces the morphism C,(f) : C,(X:,L·) —>
C.(Y; k) defined by C.(/)a = foa; we write #.(/) for H(&.(/)). In particular,
for the constant map ?' —> pt we may identify
C.(const) = ? : C„(X;k) —> k.
This is called the augmentation of C»(X;k).
Remark CS.(-) vs C.(-).
Most presentations of singular homology use CS.(-) since it is simpler to
describe, and gives the same answer. For us, however, the property C*({pt}; Ik) =
L· turns out to be really useful.
The constructions that follow are all made first at the level of 5«(-Y), then bi-
linear extension to CS»(X;Jk) and then (by factoring) in C*(X;Jc). We shall
describe only the first step, leaving the rest to the reader.
(b) Topological products, tensor products and the dgc, C*(X;k).
We recall now how topological products are modelled by tensor products of
chain complexes. As in §3, — ® — denotes — 0* —. First note that CS,(X; ]k) ®
CS*(Y;]k) is a free graded module with basis the tensor products ? ® r of
singular simplices in X and Y. Moreover C,(X:k) ? C,{Y;h) is the quotient
chain complex obtained by dividing by those ? ® r with at least one of ?, ?
degenerate. Thus, as remarked above, constructions in CS*(—) pass to C(—)
by factoring.
There are two important chain complex maps that model topological products
by tensor products. The first is the Alexander-Whitney map
AW : C._(X ? Y; k) —»¦ C.(X; k) ® C.{Y\ Ik).
defined as follows: If (?, r) : ?? -> ?' ? ? is any singular simplex on ? ? ?
then
?
AW (?, ?) = Y^ao(e0,...,ek)®To (ek,-..,en).
?·=0
The second is the Eilenberg-Zilber map
EZ : C.{X;h) ®C.{Y;h) —»¦ C,(X ? Y;k) ,
54
4 Singular chains, homology and Eilenberg-MacLane spaces
whose construction is based on a combinatorial description of the product ?? ?
?9 of two standard simplices with vertices vo,...,vp and wo,...,wq. When
? = q = 1 this reduces to the decomposition of / ? / into two triangles:
?' = ((vo,wo),(vo,wi),(vi,wi))
In general ?? ? ?9 decomposes as the union of all the linear (p + ^)-simplices
? of the form
? = ((va@),W?@) ),- ¦ ¦ ???(?)???(?))? ¦ ¦(va(p+q),W?(p+q))), D-3)
where for each i either
a(i + 1) = a(i) + 1
i + 1) = ?(i) + 1
or else
Putn(w)= ? [?(i + l)-?(i)][a(j + l)-a(j)}.
0<i<j<p+q
The idea of EZ is that if ? 6 SP(X) and r 6 5,(?) are respectively a p-
simplex and a ^-simplex then the basis element ? ® r in Cp(Jf) ? C9CK) should
correspond to the map ? ? r : ?? ? ?9 -> ? ? }'. Thus while the fact that
?? ? ?9 decomposes as the union of the ? motivates us, we do not actually need
it. We simply write down the chain
where the sum is over all ? of the form D.3). Notice that if ? or ? is degenerate
then so is CS,(a x r)(cp>9), and so it represents zero in C*(X ? ?). Thus we
may define EZ by
Straightforward (but often tedious) calculations establish the properties below
of AW and EZ. Let px : ? ? ? -> Ar and py : ?" ? F -> 7 be the projections.
We identify X = ? ? {pi} and p-Y with id ? const. : Ar x F —> ? ? {??}. On the
algebraic side we identify C.(A; A) ® C.({pi}; Jb) = C.(X; Jb) ® Jfc = C.(A; A).
Homotopy Theory 55
• [118] AW and ? ? are morphisms of chain complexes, natural in X and in
Y. D.4)
• In particular.
(id ® ?) ? AW = Ct(px) and (? ® id) ? AW = C.(py);
while D.5)
C*(px) oEZ = id® ? andC,(pY) ? EZ = ?
When X = {pt}, AW and EZ both reduce to the identity map ofC*(Y; Jk).
A similar assertion holds when Y — {pt}. D.6)
[118] Associativity For any three spaces ?,?,?,
(AW ® id) ? AW = (id ® AW) ? AW, and
D.7)
EZ ? (EZ ®id) - EZ ? (id® EZ).
as maps between C.(X xYxZ; k) and C.(X; Jk) ® Ct(Y; k) ® C,(Z; k).
[118] Interchange of factors For topological spaces X,Y and graded mod-
ules M,N define ? : ? ? ? —> ? ? X by 9(x,y) = (y, x) and 0aig :
M ®N —> N®M by 0aig(m ® n) = (-l)desm des"n ® m. Then
C,@)oEZ = ?Zo0alg : C.(X;k)®C,(Y;k) —> C,(Y xX;k). D.8)
[49] Compatibility The following diagram commutes
C.(XxX';k)®C.(YxY';k) > C.(X;k)®C.(Y;k)®C.(X';k)®C.(Y';k)
EZ EZ®EZ
C.{XxX'xYxY'\k) > C.(XxY;k)®C.(X'xY';k)
A\VoC.(idxexid) ,. ,
D.yj
where ? = zc?<8>oaig ® id.
Finally we have
Proposition 4.10 AW and EZ are inverse chain equivalences. In fact, AW ?
EZ = id and EZ ? AW is naturally homotopic to the identity.
-proof; The first assertion is a simple computation, depending on the fact that
we have divided by the degenerate simplices. For the second, we have to construct
h : Cn(X ? Y; k) —> Cn+1(X ? Y; k), natural in A' and }', such that EZoAW-
id = dh + hd. We may set h = 0 in Co (? ? ?). Suppose for some ? > 1 that h is
constructed for i < n. Let ?1?? : ?? —>?"??" be the diagonal, regarded as a
singular ?-simplex. Then ? = (EZ ? AW)(Atop) - ?1?? - hd(iltop) is a cycle in
Cn(An ? ??; k). Since ?? ? ?? is contractible we may find cn+1 G Cn+\ (?? ?
56 4 Singular chains, homology and Eilenberg-MacLane spaces
??; A-) so that dcn+\ = ?. Now for any ?-simplex (?, ?) : ?? —> ? ? ? define
/?(?,?) = C.(axr)(cn+1). ?
The Alexander-Whitney map defines a natural differential graded coalgebra
structure in C,(X;k) via the topological diagonal, ???? : X —> ? ? ?, ? 4
(x,x). The comultiplication is given by
A = AW ? C.(Atop) : C.(X;k) —»· C.(X;k)®C.(X;k).
Thus for each ? G Sn(X),
?
?(?) = AW (?, ?) = ]??? < e0, ·. · ,efc > ®?? < e*.·,... ,en > .
The augmentation is defined by
? = C,{const.) : C,(X;k) —> k = C,({pf}; *).
The associativity of AW and its compatibility with ? (cf. (b) above) imply that
(?,(?;*),?,?) is a dgc.
Moreover any ? € X may be regarded as a zero-simplex in Co(X;k) and.
clearly ?? = ?®?. Thus 1 i-j· ? defines a co-augmentation in the dgc, C,(X\ Jk).
(c) Pairs, excision, homotopy and the Hurewicz homomorphism.
For A C ?' we put
C.(X,A;k) = C4Xik)/C,(A;ls)i
it is it-free on the non-degenerate simplices of X whose image is not in .4. Its
homology, H, (X, A; Ik) is the ordinary relative singular homolog)'.
For ? C A C X we have the short exact sequence
0-+C.(A,B;le) —> C,(X,B;k) —>C,(X,A;k) —> 0
induced by the obvious inclusions; it leads to a long exact homology sequence
>Hi(A,B;k) -^Hi(X,B;k) -^Hi(X,A;k) ? Hi-i(A,B;k) —>...
D.11)
d is called the connecting homomorphism. Since H,(Y;k) = ?,{\\?;??) this
reduces to a more familiar long exact sequence when ? = ?.
If W C .4 C X has the property that the closure of W is contained in the
interior of .4, then the excision property [142] states that inclusion induces an
isomorphism
H,{X - W,A - W; Jk) ? H.(X,A;k).
The morphisms EZ and AW of (b) above factor to give (relative) Eilenberg-
Zilber and Alexander-Whitney morphisms
EZ
C. (X, A; k) ® C. (Y; k) } » C. (X x ?, ? ? ? ; k)
AW
Homotopy Theory 57
satisfying AWoEZ = id and EZoAW ~ id. Thus these are quasi-isomorphisms.
Suppose now that ? : A' x / —> Y is a homotopy from ?0 to ?\. Define
h : Ci{X;k) —»¦ Ci+1(Y;k), i > 0, by ?(?) = (-?)'?(?) ? ??(? ® id/), where
the identity map of /, id/, is regarded as a singular 1-simplex. Then
C.(<p1)-C.(<p0)=dh + hd;
i.e., ? is a homotopy from C,(<po) to ?,(?\). In particular ?*(??) = ?*(?0).
As an example, let <9?? be the boundary of ??, and regard the identity map
?? -^ ?? as a singular simplex. It represents a cycle, zn e G?(??,<9?"; Jfc),
whose homology class will be denoted by [??].
Lemma 4.12 For ? > 0, ?* (??, <9??; Ik) is a free module concentrated in de-
gree ? with single basis element [??]
Hf(An,dAn;k) = 1c ¦ [An].
proof: We may suppose ? > 0. Regard ?"" = Im(e0,..., e„_i) as one of the
faces of ?? and let L be the union of the other faces. Then L is contractible to
en. Hence H,(L;&) ? H.(An;Jk), and the long exact sequence for ? C L C ??
implies that #.(??, L; h) = 0. Thus the long exact sequence for L C <9?? C ??
provides an isomorphism
3:?4??,3??;&) A H,-.i(dAnt L; k).
On the other hand, shrinking the last coordinate shows that the inclusion
(??~1,???~1) —> C?? - {en},L - {en}) is a homotopy equivalence. This
yields
H.{dAn,L\k) \?^.?? H,(dA" - {en},L -{en};*) - ~ /f. (??~?, 8?11"'; Jfc)
Combined with the isomorphism above this gives an isomorphism
H,(An,dAn;]k) 9?H.-1(An-1,dAn-1\k),
which carries [??] to (—l)"^"]. The lemma follows by induction on n. ?
It follows from Lemma 4.12 and the long exact sequence for ? c <9?? C ??
that for ? > 1, H,(dAn;]k) is a free .fc-module on two generators
dAn] D.13)
where [eo] is the homology class of the 0-simplex eo and [<9??] is the homology
class of the cycle zn_! = Yl(-\)i{eQ...ei...en). Since (??,???) is homeo-
morphic to (Dn,Sn~1) we conclude
| JL, ? .V-,
U inn en—1. n-\ _ ) ¦"*·' l ~ n
tii{L) ,O ,Jh,) — < _ ,
' [0, otherwise
and D.14)
0, otherwise.
58 4 Singular chains, homology and Eilenberg-MacLane spaces
We use a different calculation to fix a generator in Hm(Sm;Z) = Z, m > 1.
Recall that Im denotes the m-cube. In Example ?, §1 we constructed homeo-
morphisms
Im/dlm A Sm and dlm+1 A 5m ,
and so D.14) implies that Hm(Im,drn;Z) ?? Z. Choose generators [/m] G
Hm(Im,dIm\Z) as follows. For m = 1 let [/] G Hi(I,dI;Z) be the class rep-
resented by the identity map ? of / (i ; t >->¦ t), which is indeed a relative cycle.
For m > 1 let [/m] be the class represented by ? ? {? <g> · · · <g> ?). Then [Im]
is a generator of Hm(Im.,dIm;Z) and 9[/m+1] is a generator of Hm(dlm+1 ;Z).
A straightforward calculation shows that the homeomorphisms above send [/m]
and d[Pn+l} to the same generator, [Sm] G Hm(Sm:Z).
Definition [Sm] is called the fundamental class of Sm class of Sm.
Next, recall that Hm(<p) ¦ Hm(Sm;]k) —> Hm{X;h) depends only on the
homotopy class of tp : Sm —> X. Thus set maps
hurx : Tim(X, x0) —> Hm(X; Ik), m > 1,
are defined by /iurx[y] = ifm((p)[5'71]. A straightforward computation shows
that these are group homomorphisms; and it is immediate from the definition
that they are natural; if / : (X,Xo) —> (Y,yo) is continuous then
= hury- °?*(/)·
Definition The Hurewicz homomorphism for X is the homomorphism
(d) Weak homotopy equivalences.
We shall rely heavily on
Theorem 4.15 If ? : A' —>¦ Y is a weak homotopy equivalence then C»(y) :
C»(X;Jk) —> C*(Y; Jk) is a quasi-isomorphism.
proof: Recall the face maps Aj = (e0 ... et... en) : ??-1 —> ?". We first
observe that for each ? > 0 and each ? : ?11 —> Y we can associate ?' : ?? —> ?
and a homotopy ?? : ?? ? / —> Y from ? to ? ? ?' such that
?' ? Xi = (? ? Aj)' and ?? ? (?* ? id) - ????,., 0 < i < ?
D.16)
Indeed we proceed by induction on n. Conditions D.16) define ?' on <9?? and
?? on 3?? ? /. Now the extension to ?' and ?? is just the Whitehead lifting
lemma l.o.
Homotopy Theory 59
Define a linear map / : CSm{Y\k) —> CSm(X;Jk) by ? ^ ?'. The conditions
above imply that / is a chain map and that CSm(ip) ? / — id = hd + dh, where
h(a) = CS.($G)oEZ(a®idI).
A second application of the Whitehead lemma gives, for each r : ?" —> X,
a homotopy ?? : ?? ? / —> X from (? ? ?)' to ? such that ?? ? (\t x id) =
????,, 0 < ? < ?. This then implies that / ? CSm{ip) — id — dk + kd. Hence
CS*(ip) is a chain equivalence and ?.(?) is a quasi-isomorphism. ?
(e) Cellular homology and the Hurewicz theorem.
Let (A', .4) be a relative CW complex with ?-skeleton Xn, so that Xn =
A'n_i U/n ( 1I-DS )- Let oa be the origin (centre) of D%. Put U = Xn - Ui0»)
and ? = U (?? - {oa}). Since Dn- {0} = Sn~l ? @,1] ~ Sn~l x {1}, it follows
?
that the inclusions A'n_i —> U and U^a —> ? are homotopy equivalences.
?
Thus in the diagram
C.(X„,U) C.(.Y„ -.?,-,^-^-,)
c
where C»(—) stands for C«(—;Jk), the horizontal arrows on the left are quasi-
isomorphisms, while the horizontal arrows on the right are quasi-isomorphisms
by excision. Hence the characteristic map induces an isomorphism in homology:
By D.14) we may identify H,(Xn,Xn-i;lk) as a free module concentrated in
degree ? with basis {cQ} indexed by the ?-cells of X. This implies via an easy
•induction that H,(Xk,Xr;lk) is concentrated in degrees i 6 (r,k\. Since any
singular simplex of Ar has compact image it lies in some Xk, and so any element
of C,(X;Js) is in some C,{Xk\ le)- It follows that
Hi(XtXr-tl:)=0t i<r. D.17)
Denote the free module. Hm(Xn,Xn-\\h) by Cn. Associated with the inclu-
sions Arn_i c Xn C Arn+1 is a long exact homology sequence, (cf. D.11)) with
connecting homomorphism d : Cn+i —> Cn. An easy computation shows that
ooo = 0. Thus (C = {Cn}n>o,<9) is a chain complex. It is called the cellular
chain complex for the relative CW complex, (Ar, .4).
Definition A cellular chain model for (X, A) is a morphism
m: (C,d) -*Cm(X,A;]k)
60 4 Singular chains, homology and Eilenberg-MacLane spaces
between the cellular and normalized singular chain complexes, restricting to mor-
phisms m(n) : (C<n,d) —> C.(Xn,A;k) and such that the induced morphisms
rh{n) : (Cn,0) = (C<n/C<n-i,d) —> C.(Xn,*n-i;*)
induce the identity map Cn = H»{Xn. Xn-i\ k).
When ,4 = 0, m : (C,d) —> C*(X]k) is called a cellular model for the CW
complex X.
Theorem 4.18 (Cellular chain models) Every relative CW complex (X, A)
has a cellular chain model m : (C.,9) —> C*(X,A;k), and m and each m(n)
are always quasi-isomorphisms.
proof: We construct the morphisms m(ri) inductively and observe in passing
that they are quasi-isomorphisms.
Suppose by induction that m(n) is constructed; we extend it to
m(n + 1) as follows. Represent a basis element ca G Cn+\ by a cycle za G
Cn+i(Xn+i,Xni&), and lift za to a chain wa € Cn+\(Xn+i', k)¦ Then dwa is
an ?-cycle in C,(Xn;k). Since m(n) is a quasi-isomorphism there is a cycle
ua G Cn and a chain aa G Cn+i(Xn',k) such that m(n)(va) = dwa +daa- Since
H(rh(n)) is the identity, it is immediate that va = dca. Extend m(n) to a mor-
phism m(n + 1) by setting m(n + l)(ca) = wa + aa. Since wa + aa also projects
to za, rh(n + l)(ca) = za and H(fh(n + l))ca = [za] = ca, as desired.
Finally, since H(m(n)) and ?(??(? + 1)) are isomorphisms so is
H(m(n + 1)) by the five lemma C.1). The sequence m(ri) so constructed defines
a cellular model m : (C„, <9) —> C, (X, A; Jk). Moreover for any cellular model we
have, in the same way, that each m(n) is a quasi-isomorphism. Formula D.17)
identifies Hi(m(n)) with Hi(m) for i < n; hence m is a quasi-isomorphism. ?
Remark Suppose ? : (X, A) -> (Y, B) is a cellular map of relative CW
complexes, so that it restricts to maps ip(n,k) : (Xn,Xk) -> (Yn,Yk)· Put
fn = ??(?(?,? ~ 1)) : Hn(Xn,Xn-i; *) -> ??{??,??-?-?)- It is immediate
from the definition of d as a connecting homomorphism that
/ = {fn}: (C?,d) -» (CY,d)
is a morphism from the cellular chain complex of (X, A) to the cellular chain
complex of (Y, B).
Now suppose that mx : (C?,d) -^> C,(X,A;k) and mY : (C?,d) -=*
C*(Y,B;k) are cellular chain models. We construct linear maps hn : C* ->
Cn+i{Yn;k) so that
mY f -C, (?)??? = dh + hd : (Cx, d) -> C, (Y, B; Je);
i.e., mYf is chain homotopic to Ct(<p)mx.
Homotopy Theory 61
In fact, set /i_i = 0 and assume hi constructed for i < ? so that the equation
above holds in Cxn. Let ca be a basis element of Cx+l. Then
d(myf - C,(<p)mx - hd)ca
= (mYf - C. (?)??? - dh - hd)dca
= 0.
Thus za = (mYf — Ct{tp)mx - hd)ca is a cycle in Cn+1 (Yn+X\]k). Now hdca G
h(Cx) C Cn+1 (Yn; k). It follows that za projects to the cycle (my f-C,(tp)mx)c0
in Cn+\ (??+?, ^n,' -fc)· It is immediate from the definitions that this relative cycle
is a boundary, so that
za = dxa+ya, xa G Cn+2(Kn+1;$;), ya G Cn+i(Yn; A·).
Now ?/? is an (n + 1)- cycle in Yn. Since i/n-n^n;!:) = 0 by the Cellular chain
models theorem, ya = dwa, some wa G Cn+2(Yn+\]E:). Define hn+i by setting
hn+l(Ca) = Wa-
Theorem 4.19 (Hurewicz) Let (?,??) be a pointed topological space for which
???(?,??) =0, i <r.
(i) If r = 0 (i.e., X is path connected), then the homomorphism
hurx : ?? (X, x0) —> i/j (Ar; Z)
is surjective and its kernel is the subgroup of ?? generated by the commu-
tators ??? ?~?.
(ii) Ifr>\, then Hi(X;Z) = 0, Ki <r and
hurx : ??+? (Ar, x0) —> i/r+1 (?"; ?)
is an isomorphism.
proof: The proof of Theorem 1.4 provides a weak homotopy equivalence
?: (Y,yo) —» (Ar,x0)
in which ? is a CW complex, YT = y0 and ?'r+1 = Va5^+1. Thus Theorem 4.15
asserts that Ht (?) is an isomorphism. By the naturality of the Hurewicz ho-
momorphism it is sufficient to prove the theorem for Y. Moreover, again by
naturality, it is sufficient to prove the theorem for the finite subcomplexes of Y;
i.e. we may assume 1' itself is finite.
We first consider the case r > 1 and observe that the Cellular chain models
theorem above shows that Hi(Y:Z) = 0, 1 < i < r. Moreover, since 5r+1 =
*Uer+1; l\Sa+1 is a CW complex with Yr+1 as its (r + 1) skeleton and the
62 4 Singular chains, homology and ????????^-????^??? spaces
next smallest cells of dimension 2r + 2. Thus (since r > 1) there are no r + 2-
cells, and so the Cellular approximation theorem 1.1 implies that 7rr+1(l'r+1) -=4
Kr+i(Y\Sa+l). By Hopf's theorem 1.3, this identifies ???+?(??+?) as a free abelian
?
group with basis {[??]} where ?? : S?+1 —> Yr+i is the inclusion. Hence
l/,_ l'y \ » TJ /¦y · ? \
is an isomorphism, hur' denoting the Hurewicz homomorphism for Yr+\.
Let ? : ir+i —> Y be the inclusion. Since H(Y,Yr+i;Z) vanishes in degrees
k < r + 1 (cf D.17)), Hr+i(X) is surjective. Now the commutative diagram
7??+?(??+?)-^??+1(??+1;?)
shows that Ziury is surjective.
Write Yr+i = Yr+\ U (U^e^+2) with attaching maps //? : S?+l —> }'?+?· Let
(C, <9) be the cellular chain complex for Y. If C? ? Cr+o is the basis element
corresponding to e^+2, then a straightforward computation, using the definition
of d as a connecting homomorphism, shows that dc? = Hr+\(f?)[S?+1]. Thus
it follows from the Cellular chain models theorem that the kernel of Hr+i(\) is
spanned by the classes Hr+i(f?)[Sp+i].
Now suppose g : (ST+1,*) —> (Y>yo) represents an element in kerhury. By
the Cellular approximation theorem, g is based homotopic to h : Sr+1 —> Yr+i;
i.e., [g] = ??+1(?)[/?] and 0 = Hr+1(X) hur'[h}. Thus
hur'[h] = ? hHr+i(f?)[Sr0+1] = Y^k? hur'[f?].
? ?
Since hur' is an isomorphism,
But f? is the attaching map for a cell in Yr+o, and so 7rr+i(A)[/^] = 0. It
follows that [g] = ??+?(?)[/?] = 0 and so hur is injective.
In the case r = 0, Yi = Va5^ and the van Kampen theorem [68] implies that
??A?) is generated by the circles S?. These same circles form a basis of the
free abelian group ??(??;?), and it follows that ker hur' is generated by the
commutators. Now simple modification of the proof for r > 1 completes the
argument. D
(f) Eilenberg-MacLane spaces.
An Eilenberg-MacLane space of type (?,?), ? > 1, consists of
Homotopy Theory 63
• A path connected, based space (X,Xo) such that ??(?.??) = 0, i ? ?,
together with
• A specified isomorphism of ??(?,?0) with a given group ?.
If X is a CW complex it is called cellular Eilenberg-MacLane space. Eilenberg-
MacLane spaces are often denoted by ?'(?,?). Here we shall need two simple
properties.
Proposition 4.20 Suppose given an (n—1)-connected based CW complex (X, x0),
an Eilenberg-MacLane space (?(??, ?), *) and a homomorphism ? : ??(?', Xo) —>
?. Then there is a unique homotopy class of maps
such that nn(g) = ?.
proof: The proof of the Cellular models theorem 1.4 shows that (X,xq) has
the weak homotopy of a CW complex with a single 0-cell, no cells in dimension
i, 1 < i < n, and all cells attached by based maps (Sk,*) —> (Xk,xo)· Since
weak homotopy equivalences between CW complexes are homotopy equivalences
(Corollary 1.7) we may assume (?,??) itself satisfies this condition. Thus Xn =
VaS2, and Xn+1 = Va52 U, (Up Dn0+l).
Now 5J represents a class ?? e ??(?, xq); choose gn : (VaS2, xo) -> (?(?, ?), *)
so that gn restricted to S? represents a(-ya). If ? : Xn —> X is the inclusion
then Trn(gn) = ????(?), and it follows that gn ° f '¦ (S?,*) —> (?(?,?),*) is
null homotopic. This permits us to extend gn to a map gn+\ : Xn+i —> ?(?. ?).
Extend gn+\ to the rest of X inductively over the skeleta, using the fact that
??(?(?,?)) = 0, i > n.
Given a second such map h we use the fact that ??(/?) = Kn(g) to conclude
that hn ~ gn : (Xn,x0) —> (?(?,?),*). Extend the homotopy ? inductively
over the skeleta: if ? is defined in ?'?.· ? / and Dk+l is a (k + 1) disk attached
by Sk —->· Xk then ? yields a map Sk ? / —-> ?(?,?). Using h in Dk+l ? {0}
and g in ?>fc+1 ? {1} we extend to a map d(Dk+1 ? /) -» ?(?,?). Since k >
n> ^fc+i (?(?, ?)) = 0 and this map extends to all of Dk+1 ? /, thereby extending
? to Xk+i x I. ?
Proposition 4-21 Suppose ? is an abelian group. Then there exists ? ?(?,?)
and any two have the same weak homotopy type.
proof: Suppose ? > 2. Write ? as the quotient of a free abelian group on
generators ga divided by relations r0. The proof of the Hurewicz theorem D.19)
identifies ??(??52) = ?? Z9a- Let f3 : E?,*) —> VaS2 represent r?. Then
the proof of the Hurewicz theorem also identifies Kn(VaSSLI{fg} UpD0+1) = ?·
Put Yn+1 = Vu52 U{fg} \}? ?>3+1· Now create Yn+2 by adding (n + 2) - cells
to ^n+i to kill 7Tn+1(yn+1) and continue this process inductively, creating Yk+\
64 4 Singular chains, homology and Eilenberg-MacLane spaces
by adding (k +1)- cells to Yjt to kill ?^??· The Cellular approximation theorem
1.1 will show that Y is a ?(?,?).
For ? — 1 we simply note that, since path spaces are contractible, the long
exact homotopy sequence B.2) for the path space fibration implies that ?,?(?, 2)
is a ?(??, 1).
Let (?',??) be a cellular model for an Eilenberg-MacLane space (Y, y0) and
suppose ?(?,?) is any Eilenberg-MacLane space of the same type. Proposition
4.20 gives a map g : (?.,??) —> ? (?,?) such that irn(g) is the identity map of
?·. Thus ? is a weak homotopy equivalence. D
Remark When ? = 1, ? (?, l)'s exist for any group ?, and are constructed in
the same way: ?? is the quotient of a free group on generators ga by relations rp,
and adding corresponding 2-cells to VS^ gives a space with ?? = ??. For details
see [68].) We will not carry this out since such K(ir, l)'s do not arise in this text.
Exercises
1. Compute the homology with ? coefficients of the space obtained from the
sphere 5" by attaching an (n + l)-cell along a continuous map of degree ? and
another (n + l)-cell along a continuous map of degree q with ? and q relatively
prime.
2. Suppose that Ik is a field. Compute the algebra H*(SP x5«x Sr;Ik) for
1 <p<q<r.
3. Let X be a finite complex and Ik a field. Prove that dimii»(Ar, Ik) < co.
Using §3-exercise6, prove that ?(?) = ^ (-l)dim ? . Compute ?(?') when
X = Sn ,RPn ,CPn,T-.
4. Let X(n) be obtained by attaching to a space ?" cells of dimension n + 2 and
higher to kill off the homotopy groups of A" in dimensions above n. Prove that the
homotopy fibre of the canonical map ?^?+1> -> A"(n) is an Eilenberg-MacLane
space ? (??+1 (?),? + 1).
5. Prove that the cap product, CP(X; Ik)®Cg(X; Ik) -> Cq-p(X; Ik) , /<gc ^ /?
c = (l®f)AW(c) induces a natural of C*{X; Jfc)-module structure on C*(X;Ik).
5 The cochain algebra C*(X]k)
As usual, we work over an arbitrary commutative ground ring, k.
Recall from §3 that given two complexes (M,d) and (N,d) we can form the
complex (Hom(M,N),d). with d(f) = df - (-l)dezffd. In particular, the nor-
malized singular cochain complex of a topological space X is the cochain complex
C*(X;k) = Eom(C*(X;k),k).
Thus Cn(X;k) = Horn (Cn(X;k),k) and d{f) = -(-\)^&ffd. In particular,
an element / 6 Cn(X; k) may be thought of as a set theoretic function / :
Sn{X) —> k vanishing on the degenerate simplices, and
n+l
(d(f))(a) = Y{-l)d*zf+i+lf{ao<e(u...,ei,...,en+l >).
i=0
The augmentation ? : C0(X;k) —> k may be regarded as an element 1 6
..C°(X;k).
Recall from §4(b) that the Alexander-Whitney comultiplication in
C,{X) k) is defined by ? = AWoC*{Atop) ¦ C.(X; k) —> C,{X; k)®C*(X; k).
Dually, as described in §3(d), C*(X; k) is a cochain algebra whose natural asso-
ciative multiplication, the cup product, is defined by
= (-l)k("-Vf(ao(e0,...,ek))g(ao(ek,...,en)),
f ? Ck(X;k), g ? Cn~k(X;k), ? € Sn(X).
Definition The cochain algebra C*(X;k) is called the normalized singular
cochain algebra of X. The cohomology algebra H (C* (X; $:)) is denoted H* (X; k)
and called the singular cohomology of X.
If ? : X —> Y is a continuous map then we put ?*(?) = Horn (C, (?); k) :
C*(Y;k) —> C*(X;k). Just as ?,(?) is a morphism of differential graded
coalgebras so C*(<p) is a cochain algebra morphism, and ?*(?) = ? (?*(?)) is
a morphism of graded algebras.
If .4 c X then C*(X; k) —> C*(A;k) is surjective because (as graded mod-
ules) C.(A;Jk) is free on a subset of a basis of C,(X;k). The kernel of this
restriction, C*(X, A;k), is an ideal in C*(X;k) and the short exact sequence
0 —>· C*(X,A;k) —> C*(X;k) —> C'(A;le) -4 0
gives rise to a long exact cohomology sequence. Note that C*(X,A;k) con-
sists of the functions S*(X) —> k that vanish on degenerate simplices and on
simplices in A. Thus we may identify the cochain complexes C*(X,A;k) and
66 5 The cochain algebra C*(X;k)
Horn (C»(X, A; k),k). We call C*(X,A;k) the complex of normalized relative
singular cochains; H*(X,A;k) = H (C*(X, A; He)) is the relative singular coho-
mology.
Left and right multiplication by C*{X;k) make C*(X,A;k) into a left and
right C*(A',-l;)-module. Thus H*(X,A;k) is a left and right ii*(Ar,-ifc)-module.
Let / ? Cn(X,A;]k) and ? € Cn(X,A;k) be respectively a cocycle and a
cycle. Then f(z) depends only on the cohomology class [/] and the homologv
class [z\. Define
a :H*{X,A:k) —> Horn (Hm(X, A; k),k) E.1)
by (a[/]) [z] = f(z). This defines a pairing between H* and H» that we denote
by ([/], [z]}, in line with the convention in §3(a).
Next, let ? : (?, ?) ? Y —> (X, A) and q : ? ? ? —> Y be the projections.
Since ?* ((?,?) ? Y;k) is a right H*(X ? Y; J:)-module, a linear map
? : ?*(?, .4; Jfc) ® H'(Y; k) —> ?* ((?., ?) ? Y; k) E.2)
is defined by ? ([/] ® [g]) = ii*(p)[/] · H*{q)\g].
Proposition 5.3 (i) If k is a field then a is always an isomorphism and so
{ , } is non-degenerate. In particular, H*(X,A;k) has finite type if and only if
H*(X,A;k) does.
(ii) If k is a field and at least one of H„(X,A;k), Ht(Y;k)
has finite type then ? is an isomorphism.
proof: (i) This is just the assertion of Proposition 3.3 that "H" commutes
with "Horn".
(ii) As follows from §4(b), the Alexander-Whitney map is a quasi-
isomorphism C*((X,A) xY;k) A C*(X,A;k) ®C*(Y;k). Since homology
commutes with tensor products (Proposition 3.3) we obtain an isomorphism
if, ((?, ?) ? Y; k) A H, (X, A; k) ® ?, (?; k).
Because we have supposed one of H,(X, A; k), H*(Y; it) to have finite type, we
may identify H*(X,A;k) ® H'(Y;k) as the dual of H,(X,A;k) ®H*(Y;k),
and ? as the isomorphism dual to the isomorphism above. D
Finally, if A C ? C X then the short exact sequence
0 —>· C*{X,B;k) —> C*(X,A;k) —> C*{B,A;k) —>¦ 0
leads to a long exact cohomology sequence dual to D.11).
Homotopy Theory 67
Exercises
1. a) Prove that if A C A' and ? C A' then the cup product C(X;Ik) ?
C*(X;Ik) -> C"(X;Ik) restricts to a cup product C*(X, A; Ik)®C*(X,B;Ik) ->
C*(X,AUB;Ik) and thus induces a cup product H*(X, A, Ik) ®H*(X,B; Ik) ->
H*(X,AUB;Ik).
b) Prove that if .4 and ? are contractible open sets such that A' = Al)B then
for any ? > 0 and q > 0, U : #P(A; a) ® if«(A'; ft) -> Hp+q{X, Ik) is zero
c) Prove that a suspension (reduced or not) has a trivial cohomology algebra.
d) Prove that CP2 and S2 V S4 do not have the same homotopy type.
2. Suppose that A is a field Prove that the morphism ?, defined in 5.2, is an
isomorphism of algebras when ^1 = 0 Compute the algebra H*(X;Ik) when.
X = S1 ? S1 ? ... ? S1 (?-times), A' =7vZ, A' = (S3 V S7) ? E5 V S9),
X = F ? ?.
3. Prove that, for a 6 tt6(CP2 V S2), the spaces ??? = CF2 V S2 Ua O7 have the
same cohomology algebra.
4. Prove that ii dim Hi (X; Ik) = oo, then H1(X:Ik) is uncountable.
6 (R, (i)-modules and semifree resolutions
As usual, we work over an arbitrary commutative ground ring, Ic. Thus graded
module means graded Ik-module, linear means It-linear, and Hom(—, —) and — ®
— stand for ?????.(—, —) and — <8>?· — ·
Our focus in this section is on modules (M, d) over a dga (R, d) as defined in §3.
While the emphasis is on left (.ft, demodules the results apply verbatim to right
modules. In the classical context of modules M over a ring R (no differentials)
it is standard to construct a quasi-isomorphism (P*,d) -^ (M,0) from a chain
complex of free .ft-modules to M; these are the free resolutions of M.
Free resolutions were generalized by Avramov and Halperin[16] to modules
over a dga (R,d). Their analogue of the complexes F» are the semifree (R,d)-
modules defined below, which have these two important properties:
• Any (R,d)-module admits a quasi-isomorphism from an (R,d)-semifree
module.
• Any morphism from an (R, d)-semifree module lifts (up to homotopy) through
a quasi-isomorphism.
Thus (cf. introduction to §1) semifree modules over (.ft, d) are the exact analogues
of CW complexes.
This section is organized into the following topics:
(a) Semifree models.
(b) Quasi-isomorphism theorems.
(a) Semifree models.
We begin with some basic definitions. Recall the tensor product ? ®r M
and the module of A-linear maps ?????(?, ?') defined in §3(b) for any right
A-module ? and left A-modules M and M', If (N,d),(M,d) and (M',d) are
(R, ^-modules then (cf. §3(c))
r M,d) is a quotient complex of (N, d) ® (??, d), and
• (HorriR(M,M'),d) is a subcomplex of Hom(M, M')-
A morphism ? : (M,d) —i- {M',d) is an A-linear map of degree zero, sat-
isfying ?? = ??. Two morphisms, ?, ?, are homotopic if ? — ? = ?? + Bd
for some A-linear map ?;? is a homotopy and we write ? ~? ·?. Thus mor-
phisms are precisely the cycles of degree zero in UomR(M,M') and two mor-
phisms are homotopic if and only if they represent the same homology class in
#(H(MM'))
In particular an equivalence of (.ft, demodules is a morphism ? : (M.d)
(M',d) such that for some morphism ? : (M',d) —> (M,d) we have ?? ~?
Homotopy Theory 69
and ?? ~A idj[j'. Thus an equivalence is a quasi-isomorphism. The morphism
? is called an inverse equivalence.
In general, a cycle of degree A; in HomA( A/, M') is an R - linear map / satisfying
dof= (-l)de^/od. It induces the if(#)-iinear map H(f) : H(M) —> H(M')
defined by H(f)[z] = [f(z)]. Clearly H(f) depends only on the homology class,
[/], of / and [/] !->¦ H(f) defines a canonical linear map H(HomR(M, M')) —>
HomH{R)(H(M),H(M')).
Finally if W is a graded module then unless otherwise specified R <8> W will
denote the A-module defined by ?·F<8>??>) = ab^w. When w ?-» l®w is injective
we identify W with the image 1 <g> W C R <8> W.
Definition A left (R, d)-module (M, d) is semifree if it is the union of an in-
creasing sequence
M@) C M(l) C ¦ · · C M(k) C F.1)
of sub (i?,d)-modules such that ?/@) and each M{k)/M(k — 1) are i?-free on a
basis of cycles (cf. §3). Such an increasing sequence is called a semifree filtration
of (M..d).
A semifree resolution of an (R, d)-module (Q, d) is an (R. d)-semifree module
(?/, d) together with a quasi-isomorphism
m : (M, d) A (Q, d)
of (R, d)-modules.
Semifree resolutions play the same role for modules over dga's that ordinary
free resolutions do in the ungraded case. Moreover they generalize the classical
case: if ungraded objects are regarded as graded objects concentrated in degree
zero then free resolutions are examples of semifree resolutions.
Semifree resolutions also play a quite analogous role to that of CW complexes
within the category of topological spaces, as will be illustrated shortly by re-
sults that mimic several of the theorems in §1. First, however, we make some
preliminary observations.
Remark Suppose {M(k)} is a semifree filtration of (M,d). Then Jl/@) and
each M(k)/M(k- 1) have the form (R,d) <g> (Z{k).O) where Z{k) is a free k-
module. Thus the. surjections M(k) —> R <g> Z(k) split:
M{k) = M{k-l)©{R®Z{k)), and d:Z(k) —> M(k-1).
In particular, if we forget the differentials., M = R ® ( ® Z(k) ) is a free R-
, , \A.-=0 /
module.
Lemma 6.2 // (R,d) —> (S.d) is a morphism of dga's, and if (M,d) is a
semifree (R.d)-module then (S®RM,d) is (S,d)-semifree.
70 6 (R; d)-modules and semifree resolutions
proof: Let {M(k)} be a semifree filtration for (M.d). From the formula in
Remark 6.1 we deduce
S ®r M(k) = S®r M(k - 1) © (S ® Z(k))., d : Z(k) —> 5 ®R M(k - 1).
This exhibits {5 ®r M(k)} as a semifree filtration for E ®r M.d). D
Lemma 6.3 Suppose an (R.d)-module (M,d) is the union of an increasing se-
quence M@) C ?/A) C · · · of submodules such that M@) and each M(k)/M(k-
1) is (R,d)-semifree. Then (M,d) itself is semifree.
proof: Put M(—1) = 0. In the same way as in the Remark we may write
oo
M(k) = M(k- ~
with Z(k, C) a free graded Jc-module and
d : Z(k, ?) —> M(k - 1) © I R ®
Thus M is A-free on the union {za} of the bases of the l:-modules Z(k, ?), with
Z(k, ?) a free graded ic-module and
d:Z(k,?) —
Define an increasing family W@) C W(l) C · · ¦ of free ic-modules inductively
as follows: W@) is spanned by the za for which dza — 0 and W(m) is spanned
by the zo for which dza e R ¦ W(m - 1). Then {R ¦ W(m)} will be a semifree
filtration of (M,d), provided that each za is in some W(m). Write (i,j) < (k,?)
if i < k or if i = k and j < I. If za G Z(k,t) then dza = ????? with ?? e R
and ?? e Z(i,j), some (i,j) < (A,?). We may assume by induction that each
such ?? is in some W(m.?). Put m = max^m^. Then dza e H7(m) and so
2a e I-F(m + 1). D
Let ? : (?, d) —> (Q,d) be a morphism of (i?,d)-modules, and for any third
(i?,d)-module, (M,d), denote by
) : HomA(A/,P) —> HomA(M,Q)
the morphism of complexes defined by ? ?-> ? ? ?.
Proposition 6.4 Suppose (M,d) is semifree and ? is a quasi-isomorphism
Homotopy Theory 71
(i) HomA(M, 77) is a quasi-isomorphism.
(ii) Given a diagram of morphisms of (R,d)-modules,
(Rd)
F.5)
(M,d) -+¦ (Q,d) ,
there is a unique homotopy class of morphisms ? : (M,d) —> (P,d) such
that
? ? ? ~A ?.
(ni) A quasi-isomorphism between semifree (R,d)-modules is an equivalence.
proof: (i) As remarked in Lemma 3.2 it is sufficient to show that given / e
HomA(M,?) and g € UomR(M,Q) satisfying d(f) = 0 and 77 ? f — d(g) we
can find /' e HomR(M,P) and g' e HomR(M,Q) satisfying d(f') = f and
d(g') = 77 ? /' - g. Let r = deg /.
Choose a semifree filtration {M(k)} of M, put M(—1) = 0 and, as in Remark
6.1 write M{k) = M(k - 1) ? (R ® Z(k)) where Z(k) is Jfc-free and d : Z(k) —>
M(k - 1). We construct /' and g' inductively by extending from M{k — 1) to
M(k).
For this let {za} be a basis of Z(k), put pa = f(za) - {-l)rf'(dza) and
put qa = g(za) - (-l)rg'(dza). By hypothesis the equations d(f') - f and
d(g') =770 / - g are satisfied in M(k — 1). It follows after a short calculation that
dpa = 0 and ?(??) = dqa. Since 77 is a quasi-isomorphism there are (by Lemma
3.2) elements p'a e ? and q'a € Q such that dp'a = pa and dq'a = ?{?'?) ~ Qa·
Now extend /' and g' to A-linear maps in M(k) by putting f'(za) — p'a and
9'{za) = q'a.
(ii) As remarked near the start of this section, ff0(Homfl(-. -)) is the
set of homotopy classes of morphisms. Thus by (i), composition with 77 induces
a bijection from homotopy classes of morphisms (?/, d) —> (P,d) to homotopy
classes of morphisms (M,d) —> (Q,d).
(Hi) Since (Q,d) is semifree we can find a morphism ? : (Q,d) —> (P,d)
such that 77? ~^ idc. In particular, 7]?? ~^ ????? = ????. Since (?, d) is semifree
it follows from the uniqueness in (ii) that ?? ~^ idp. G
Remark Notice that Proposition 6.4 (ii) is the analogue of the Whitehead
lifting lemma 1.5. As in §1 we use this to prove an analogue of the Cellular
models theorem 1.4.
Proposition 6.6
(V Every (R,d)-module (Q,d) has a semifree resolution m : (M,d) -^> (Q,d).
72 6 (R, cu)-modules and semifree resolutions
(ii) If m' : (M',d) -=·> (Q,d) is a second semifree resolution then there is an
equivalence of (R, d)-modules a : (M',d) —>· (M,d) such that m ? a ~h m!.
proof: (i) Let V@) be a free graded $>module whose basis is a system of
generators of the A-module of cocycles of Q. Set M@) = (R, d) ® (V@), 0). The
inclusion V@) —> Q defines a morphism g@) : (M@),d) —> (Q,d). Clearly
H(g@)) is surjective. We now construct an increasing sequence of morphisms
g(k) : {M{k),d) —> (Q,d). If g(k - 1) is defined, let V{k) be the free Jfe-
module whose basis va in degree i is in one to one correspondence with cocycles
wQ representing a system of generators of kerii,-_i(p(fc — 1)). Set M(k) =
M(k — 1) ? (R ® V(k)), and put duQ = wa. Denote g(k - 1) simply by g.
Since g{wa) is a coboundary, 5(u;a) = d(xa) we put g(k)(va) = xa. Finally, set
?/ = \jM(k).
k
(ii) Proposition 6.4 (ii) asserts the existence of a morphism ? : (M',d) —>
(M,d) such that ?? ? a ~^ in'. Thus H(m) ? ? (a) = H(m') and so ? (a) is
the isomorphism H(m') ? H(m)~l. In other words, ? is a quasi-isomorphism.
Hence, by Proposition 6.4 (iii) it is an equivalence. D
(b) Quasi-isomorphism theorems.
A basic property satisfied by semifree modules is 'preservation of quasi-iso
morphy' under the operations Horn and <8>. First we set some notation: suppose
f : M —> M' and g : ?' —> ? are ii-linear maps between left A-modules and
define
HomA(/,p) :HomA(M',P') —>HomA(M,P) by ? ^ (-l)degideg/5 ? ? ?/.
Then, if h : Q —> Q' is an A-linear map between right il-modules, define
h®Rf: Q®RM -4 Q'®RM' by /
If /, g and h commute with the differentials then Hom^(/, g) and h (g>? / are
morphisms of complexes.
Proposition 6.7 Suppose (M,d) and (M',d) are (R,d)-semifree.
(i) If f and g are quasi-isomorphisms then so is Momn{f,g).
(ii) If f and h are quasi-isomorphisms then so is h (8>h /.
proof: (i) Since (M,d) and (M',d) are semifree, Proposition 6.4 (iii) asserts
that / is an equivalence. Thus there is an inverse equivalence /' : (M',d) —>
(M, d) and ii-linear maps ? : M —-> M and ?' : M' —» M' such that
f'of- idM = ?? + ?? and / ? /' - idM, = dB' + ffd. F.8)
Apply Homfl(—,idp>) to these formulae to conclude that Romn(f,idp') is an
equivalence. Since (M, d) is semifree. Hom^(idA/, g) is just the quasi-isomorphism
Homotopy Theory 73
Eomfi(M,g) of Proposition 6.4 (i).
Thus Horrifl(/,g) = Eomn(idM,g) ° ?????(/, idp<) is a quasi-isomorphism.
(ii) Applying idc ®r — to the formulae F.8) above we deduce that idq, ®r
f is an equivalence. Since h®R f = (idci ®r /) ? (h ®r id m) it is sufficient to
show that h ®r idM is a quasi-isomorphism. Let M(k) be a semifree filtration;
it is enough to show that each h ®r idM(k) is a quasi-isomorphism, and we do
this by induction on k.
Indeed, since M(k)/M(k - 1) = (R, d) ® (Z(k), 0) with Z(k) a it-free graded
module it is obvious that /?<8>?^?/(?:)/?/(?:-?) is a quasi-isomorphism. Moreover,
as ^-modules M{k) = M(k - 1) ? {R ® Z{k)) — cf. the Remark in §6(a). Thus
the commutative diagram of complexes,
0 ^ Q ®h M(k - 1)
0 >~Q'®(i7^T)
F.9)
is row exact. Now the Five lemma 3.1. applied to the resulting map of long exact
homology sequences, gives the inductive step. ?
Remark 1 In Proposition 6.7 (ii) the identical argument shows that h ®h /
is a quasi-isomorphism if (Q,d) and (Q',d) are semifree and (M.d) and (M',d)
are unrestricted.
As it turns out, a somewhat more general result will be required. For this, fix
a dga morphism
?: {R,d) —> (S,d).
Thus any left (or right) (S, d)-module, (N, d) becomes an (R, d)-module via x-n =
?(?)?, ? € R, ? € ?.- Now suppose given the following left and right modules
over (R, d) and (S,d) and i?-linear maps f,g and h of complexes:
• / : (M,d) —> (M',d); M is left over R and M' is left over 5.
• g : (?1,d) —» (?,d); ?' is left over S and ? is left over R.
• h : (Q,d) —> (Q',d); Q is right over i? and Q' is right over 5.
Define
Hom,p(/,0) : Homs(M', P') -» HomA(M, ?) by ? .-> (-l)desi d^fgo^o f
and
h®pf: Q®RM —> Q' ® s M' by q®Rm^ h{q) ®s f(m).
Theorem 6.10 Suppose (M, d) is (R, d) -semifree and (AI1, d) is E, d) - semifree.
74 6 (R, d)—modules and semifree resolutions
(i) //</>,/ and g are quasi-isomorphisms, so is Homv,(/, g).
(ii) If ?, h and f are quasi-isomorphisms, so is h®vf.
proof: As observed in Lemma 6.2, (S ®R M,d) is E, <i)-semifree. Moreover,
since (M,d) is (R. d)-semifree
<p®Rid:(R®RM,d) —> (S®RM,d)
is a quasi-isomorphism, by Proposition 6.7 (ii). On the other hand, / extends to
the morphism of E, d)-modules
/' :(S®RM,d) —>(M',d), f'(y®Rm) = yf(m), y e S,m e M.
Clearly f = f ° (<p <8>h id) and so /' is also a quasi-isomorphism.
(i) Note that HomsE ®R M, -) = HomR(M, —) and that Hom,^/,^) is the
composite of the morphisms
uomR{idM,g) : HomA(Ai,i") —>HomA(M,P)
and
uoms(f',idp.) : Homs(M',P') —> Homs(S ®R M,P').
In view of our remarks above, Proposition 6.7 (i) implies that both these mor-
phisms are quasi-isomorphisms.
(ii) Observe that Q'®s{S®RM) = Q'®RM and that h®vf is the composite
of
idQ' ®s f ·· Q' ®s (S ®R M) —>· Q' ®s M'
with
h ®h idM : Q ®R M —> Q' ®R M.
Now apply Proposition 6.7 (ii). D
Remark 2 The argument in 6.10 (ii) shows that h ®? f is also a quasi-
isomorphism if (Q;d) and (Q',d) are semifree and {M,d) and (M',d) are unre-
stricted — cf. Remark 1, above.
Finally, we establish a partial converse to Theorem 6.10 for chain algebras.
Recall that a chain algebra is a dga, (R,d) and that R = R>0- The surjection
-Ro —> Hq{R), x ·->¦ [x\ may be regarded as a morphism
eR:{R,d) ->(Ho(R),0)
natural with respect to chain algebra morphisms.
Suppose given
• a chain algebra quasi-isomorphism ? : {R,d) —> (R',d).
omotopy Theory 75
• a right (i?,<2)-semifree module (N,d) and a right (R\ d)-semifree module
(N',d), both concentrated in degrees > 0.
• a morphism / : (N,d) —> {N',d) as above (i.e., f(nx) — /(?)?(?), ? e ?,
? ? R).
ien we may construct the morphism of chain complexes
/ ®„ ?0{?) : {N,d) ®R Ho(R) -> (N',d) ®A. Ho(R'). F.11)
ieorem 6.12 With the hypotheses above:
f is a quasi-isomorphism -?=?- / ®? ?0(?) is a quasi-isomorphism.
oof: We have only to prove <== since the reverse implication is just Re-
irk 2. Define a decreasing sequence of differential ideals
RDI1 D J1 D /2 D · · · D /n D Jn D · · ·
setting
{0, A: < ? - 1 [ 0, k <n-l
(Im d)*, jfc = ? - 1 and (Jn)k = < (kerd)fc, fc = ?
At, A: > ? { Rkl k > n.
lese constructions are clearly natural.
By inspection, H{In/Jn) = 0. Since (N,d) is semifree, ? ®r {0} -=> iV ®?
/Jn. Thus
In/J ^
n/Jn ^>
ice both sides have zero homology.
Also by inspection,
n/jn+l\ _\ Hn(R), k = ?
\
fjn/j
k \ 0, otherwise.
lus we may write ? ®r Jn/In+l = (N ®rH0(R)) ®ho(R) Hn{R)- Now
®R H0{R) —y ?' ®R' Hq{R') is a quasi-isomorphism of semifree H0(R)-
)dules. Tensoring this with the isomorphism Hn(R) -^» Hn(R') produces a
asi-isomorphism (Theorem 6.10) and so
N®r Jn/In+l ^N'®R(jy/(I')n+1.
>w an obvious induction via the five lemma shows that
TV ®r R/r A N> g,A, R'/(I')n, ? > 1.
76 6 {R, d)—modules and semifree resolutions
But since ? - N>0, the modules ? and ? ®r R/In+3 coincide in degrees
< ? + 1. Thus their homology coincides in degrees < n. It follows that H{f) is
an isomorphism. n
Exercises
1. Let V and V" be two copies of the graded vector space V and (R <g> V,d)
a semifree module over the cochain algebra {R,d). Prove that there is a (R,d)-
semifree module of the form (R <g> (V ? V" ? sV),D) such that
(i) the natural inclusions ? , i" : {R®V, d) <^->(i?<g> (V ? V"? sV), D) defined
by i'x = ?', i"x = x" are quasi-isomorphisms,
(ii) Dsv = 1 <8> v' + 1 <8> v" + S(dv), ? e V where S : R®V -) R® sV denotes
the unique extension of ? ·-» sv to an A-linear map of degree +1.
Deduce that two morphisms of i?-modules f,g : (R<&V,d) —>¦ (M,d) are
homotopic if and only if there exits a morphism of A-modules F : (R® (V ?
V" ® sV),D) -» (M, d) such that F o i' = f and F o i" = g.
2. Prove that the two morphisms of (Q[x],0)-modules f,g : Q[x] ® (QaeQo) -»
Q[s] ® Qz defined by: deg ? = 4, deg a = 2, deg 6 = 5 = deg z, da = 0 = <2z,
db = xa, f(a) = ^(a) = 0, f(b) = ? and g(b) = 0, induce the same map in
homology but are not homotopic.
3. Let Zfc be a field and (T(V),d) be a chain algebra over Ik. Construct a
contractible semifree (T(F), demodule of the form (T(V) <g> {Ik ? sV),D) with
' Dsv - ? ® 1 e TV ® sV.
4. Assume that in diagram F.5) the morphim ? is onto. Prove that there is a
unique morphism ? such that ?? = ?.
5. Let f,g : ? -> M and ? : M —>¦ iV be morphisms of A-modules. Prove
that if ? is semifree and ? is a quasi-isomorphism, then / ~# g if and only if
?°? -R ?°9-
6. Let M and IV be chain complexes over R = {R, 0) and assume that M and
H{M) are A-free modules. Prove that H{M ® N) = ii(M)
7. Let X be a finite CW complex. Prove that the cellular chain complex of
X, C?, is a semifree Z-module and that for any space Y the quasi-isomorphism
C? 4 Ct{X) extends to a quasi-isomorphism C? ® Ct{Y) 4 Ct{X) ® C,{Y).
8. Let ? : {R, d) -^ Ik an augmented cochain algebra and {P, d) an {R, d)-semifree
module. Consider the surjective cochain map {P,d) -> {Ik ®r P,d). If R° — Ik
and d = 0 we say that ? is a minimal semifree module. Prove that if R1 =0
then any {R,d)-module M, with the property that for some r0, Mr = 0, for all
r < r0, admits a minimal semifree resolution ? = {R <g> V, d) 4 M and that a
quasi-isomorphism between two minimal semifree resolutions is an isomorphism.
7 Semifree cochain models of a fibration
As usual, we work over an arbitrary commutative ground ring, Jk.
We simplify notation in this section by writing C*(—) instead of C*(—;Jk)
and H*(—) instead of H*(—;Jk). Thus (cf. §5) a continuous map f ¦ X —> Y
induces a morphism of cochain algebras C* (/) : C* (X) <— C* (?), which makes
C*{X) into a left (resp. right) C*(l')-module via a- b = C*(f)aUb (resp.
b ¦ a = b U C*(/)a). We shall also simplify notation by denoting the tensor
product over C*(Y) of C*(y )-linear maps by ? <8> ? instead of ? ®?·(?) ?-
Let F be the fibre of a fibration ? : X -> Y, as defined in §2. The purpose of
this section is to show, under mild hypotheses, how to compute the cohomology
of F from the left C*(Y)-module, C*(X). The answer is surprisingly simple: if
??? : (??,?) -?» C*(X) is a C*(y)-semifree resolution, then
as graded vector spaces. (This will be proved if Jk is a field, y is simply connected
and one of Ht(F;Jk) and Ht{Y;Jk) has finite type.)
Thus for the rest of this section we fix the following:
• A fibration ? : X —>¦ Y with simply connected base y and
fibre inclusion j : F —>¦ X at a basepoint y0 e Y.
G.1)
• A morphism of left C*(Y)-modules, ??? : (??,?) —> C*(X),
in which (MY,d) s (C*(Y) ® W,d) is C*(y)-semifree.
To state the main theorem we also require the following conventions and con-
structions dealing with pullbacks. Observe that ?? and C*(Y) <8>c-(y) ?? are
identified by the inverse isomorphisms ? t-> 1 <8> ? and ? - ? <—? ? <8> ?. Now a
continuous map
? -, ? —> y
determines three constructions associated with G.1):
• the pullback fibration ? ? : ? ? = ? ?? ? —> ?.
• the C* (?)-semifree module C*(Z) <S>c(Y) ??·
• a morphism of left C* (Z) -modules, mz : C*(Z) ®c-(Y) My —> C*(Xz) .
(This is really an abuse of notation, since these constructions depend on ? rather
than on Z.)
The first two constructions are self evident. To construct mz consider the
diagrams
C*(XZ) < ^ C*(X)
I ?
and c-(irz) c-(-r) G.2)
C(Z) +—- C'{Y)
78 7 Semifree cochain models of a fibration
in which the left diagram is just the pullback diagram of §2(a). Then mz :
a (g, v ?—). ?·?*(?)???(?),? G C*(Z),v G ??, is the unique C*(Z)-module
morphism making the following diagram commute:
C*(XZ) < ^ C*(X)
I my G.3)
C*(Y) ®c(Y) ??,
(Here as above we have identified ?? = C*(Y) <S>c~(y) ??).
When ? = {yo} the map ? in G.2) is just the inclusion j : F —> X of the
fibre. Moreover C*(y0) = Je and C*(ip) is the augmentation ? : C*(Y) —> k
corresponding to yo- Thus C*(y0) <8>c-(Y) ?? = k <8>c-(Y) ?? = k <8>c-(Y)
(C'(Y) <g> W,d) = (W,d)» and diagram G.3) reduces to
G.4)
Our main result in this section is
Theorem 7.5 Suppose ?? : X —> Y and ??? : ?? —> C*(X) are as described
in G.1). Assume k is a field and that at least one of the graded k-vector spaces
H*{Y), H*{F) has finite type. Then
??? is a quasi-isomorphism => ??,? is a quasi-isomorphism .
Remarks 1 At the end of this section we shall extend Theorem 7.5 to more
general cochain algebra functors (Theorem 7.10).
2 The reverse implication in Theorem 7.5 is also true (cf. Exer-
cise 1)
1 3 Theorem 7.5 is essentially due to J.C. Moore, and also follows
easily from a theorem of E. Brown [31]. W. Dwyer [47] has considerably weakened
the hypothesis of simple connectivity on Y.
We shall prove Theorem 7.5 using an inductive procedure involving relative
cochains. First we set some further notation. If i ¦. A —> Y is the inclusion
of a subspace we denote C*(Y, A; Je) simply by C*(Y, A); it is the kernel of the
surjection C*(i) : C*(Y) —> C*(A). In this case the pullback XA is the subspace
? (A) of X, and m^ and ??? fit into the commutative row-exact diagram
0 >¦ C*(X,XA) 2 >· C'{X) ^ C*(XA) ^ 0
? My_+C*(Y) <g> MY _^C'{A) (g> ??
C'(Y) 0®id C'(Y) C'(i)®id C"(Y)
Homotopy Theory 79
where ? and ? are the obvious inclusions. Indeed the right square commutes by
definition, and hence ??? ? (? ® id) factors uniquely through ? to define ???,?-
The exactness of the lower row follows because ?? is free as a module over the
graded algebra C*(Y).
Next, suppose A contains j/o and that ? : (?, ?,?0) —> (Y, A,yo) is a con-
tinuous map. In this case we have a pullback map ? : (??,??) —> (?,? ?)-
As above we may construct first ?? ? ¦ ? ? — C*{Z) ®c(Y) ?? —> C(Xz)
and then ??,?,?- It is immediate from the definitions that the following diagram
commutes:
C* (Xz, XB ) ?^~ C' (X, XA )
r,A G.6)
C(Z,B) ®c-(Y) MY < —C{Y,A) ®C-(Y) My,
where we have identified C*{Z,B)®C-(Z){C"{Z) ®C-(Y) ??) - C*{Z,B)®C-(Y)
??. Moreover the fibre of ?? ? at z0 is just {z0} ? F and ? restricts to the iden-
tification {z0} x F = F. Thus it is immediate from the definitions that
rnz = rnY: (W,d) —>¦ Cm(F). . G.7)
Finally we introduce the notation of k-regular : a morphism ? of cochain
complexes is k-regular if ??{?) is an isomorphism for i < k and injective for
Suppose now that we are in the situation of Theorem 7.5 and, further that:
• for some A c V, (Y, A) is a relative CW complex.
• y0 ? A and all the attaching maps have the form (S",*) —> (Yn-i>yo)',
i.e. they are based maps.
• all the cells of (Y, A) have dimension at least two.
• at least one of the graded vector spaces H*(Y,A), H*(F) has finite type.
• W = {W*}i>o and in ?? = (C'(Y) ® W,d),
d:Wn —)¦ Cm(Y) ® W<n, ? > 0.
Note that the last condition exhibits ?? as C" (Y)-semifree.
Proposition 7.8 Under the hypotheses above,
my is k-regular =? ???^ is (k + 2)-regular.
proof: We begin by establishing the proposition in four special cases.
Case 1: (Y A) = (Vuer D?, VuerS^), some ? > 2.
80 7 Semifree cochain models of a fibration
Let C*(Y,A) ®m C*{X) denote the tensor product of these two cochain com-
plexes (over k) and let C*{Y, ?)®& ?? denote the tensor product of the cochain
complexes C*(Y,A) and ??. We have used the notation - <8>? - for emphasis.
Define a commutative square of morphisms of cochain complexes.
id&rriY
C*(Y,A)®kMY
as follows: ?(? ®b) = C*(ir)a U b, and ? is the canonical surjection — ®k >
- ®c-(Y) -¦ By Proposition 3.3 we may identify ? (id ® ???) = id ® ? (???).
Since H(C*(Y,A)) = H*(Y,A) is concentrated in degrees ? > 2 (cf. §4(e)) it
suffices to prove that ? and ? are quasi-isomorphisms and that ??? is fc-regular.
A homotopy (Y, yo) x / —> (Y, yo) from the identity to the constant map lifts to
a homotopy (X, F) ? I -^ (X, F) from the identity to a map ? : X —> F. More
precisely, ? is a homotopy id ~ jg. The restriction of ? to F ? I is a homotopy
id ~ gj; i.e. ? is a homotopy inverse for j. Define ? — (? ?,? a) '¦ (?, ??) —>
(?,?) ? F by ??(?) = (??,??). By the long exact homotopy sequence ?? and
?? are weak homotopy equivalences, and so CF) is a quasi-isomorphism.
On the other hand, a commutative row-exact diagram
0 —*- C ((?,?) ? F) ^ C'(Y ? F) ^ C'(A ? F) ^ 0
h
0 -+ C*(Y,A) ®k C'(F) -^ C'(Y) ®k C'(F) -^ C'(A) ®k C'(F) -^ 0
is defined by Xi(a®b) = C*(-KL)a\jC*(nR)b, irL and irR denoting the projection
of - ? F on the left and right factors. By hypothesis either H'(Y,A) or H*(F)
has finite type. In the former case (?,?) has finitely many cells and H*(A) is
finite dimensional. Thus in either case Proposition 5.3 asserts that the ?* are
quasi-isomorphisms.
Now observe that C*F) ? ?3 = ? ? (??®?*(?)). Since ? is a homotopy
equivalence ?*(?) is a quasi-isomorphism. Hence so is ?.
To see that ? is a quasi-isomorphism we observe that because Y is contractible,
1; —> C*(Y) is a quasi-isomorphism. Since J: is a field ?? is trivially Jc-semifree
as well as C"*(F)-semifree. Hence Theorem 6.10 (ii) asserts that ? : -®kMY —>
— ®c-{Y) ?? is a quasi-isomorphism.
Finally, consider the commutative diagram
C* ( ?) ) C* ( F)
m
C'(Y)
Homotopy Theory 81
Here C"(j) is a quasi-isomorphism because j is a homotopy equivalence, while ?
and ? <8> id are quasi-isomorphisms because Y is contractible and ?? is semifree.
Since fhy is fc-regular (by hypothesis), ??? is /c-regular too.
Case 2: Y = .4U|Je2-
a
Because cells are attached by based maps the characteristic map has the form
? ¦ (Va^^Va^r1) -»· (Y,A). Put ? = \JaD2 and ? = Va-ST1. and
consider the pullback
(?,?)
Let oa be the centre of ?>? and put ? = ? - \\{oa} and U = Y - U{oe}.
a a
The inclusions ? c—> ? and A c—> U are then homotopy equivalences and so, as
in §4(e), an excision argument shows that C*(V0 is a quasi-isomorphism. Since
MY is semifree, ?'{?) <g> id : C'(Y,A) ®c-(Y) My —> C'{Z,B) ®C-(Y) My is
also a quasi-isomorphism. Moreover the inclusions ?? c—> Xo and Xa c—^ Xu
are weak homotopy equivalences so that, again by excision, C*(tp) is a quasi-
isomorphism.
Consider diagram G.6). The horizontal arrows are quasi-isomorphisms so we
need only show mz,B is {k + 2)-regular. As remarked in G.7) fnz = ???. Since
H*(Z,B) = H"(Y, A) this conclusion now follows from Case 1.
Case 3: (Y, A) has only finitely many cells.
Let Yn be the ?-skeleton of (Y, ^4), denote ??? simply by Xn and put m(n, s) =
mYntYs : C*(yn,ys) ®c-(Y) MY —)¦ C'(Xn,Xs). By Case 2, each m{n + l,n)
is (k + 2)-regular. Induction on ? via the Five lemma 3.1 and the row exact
diagram
m(n+l,n) m(n+l,O)
shows that each m(n, 0) is (k + 2)-regular. Since (Y, A) is finite ???,? — m(n, 0)
for some n. Hence ???,? is (k + 2)-regular.
Case 4: HS(Y, A) = 0, s < k + 3.
Here we show ???,? is {k + 2)-regular by proving that
Hs{C*{Y,A)®c.iY)MY) = 0, s < k + Z and Hs {C*{X,XA)) = 0, s < k + 2.
For this recall that MY = {C*{Y) <g> W,d) with W = {W'J^o and d : Wi —>
C*(Y) ® ?^<\ This gives C*(y,/1) ®c-(r) My = (C*(Y,A) ® W,d) with d :
82 7 Semifree cochain models of a fibration
Wi __,. Cm{Y,A) ® W<1. Since if5 {C*{Y,A)) = HS{Y,A) = 0, s < Jfc + 3, the
exactness of
H (Cm(Y, A) ® W<*) —>¦ H (C*(Y, A) ® T<F^) —s· ii (C*(F, A)) ® W*
implies via induction on i that #s (C*(Y, A) ® T'F^') = 0. s < A; + 3, all i. Hence
iP(C*(F,A) ® W) = 0, s < A; + 3.
Next observe that a non-zero class a in H*(X,Xa) restricts to a non-zero
class in H*{Xz,Xa) for some finite subcomplex {Z,A) C (Y,A). (Indeed (cf.
§5) ? is non-zero on some cycle ? in C*{X,XA) which, for compactness reasons,
is necessarily in some C*{Xz,Xa)·) Thus to prove the second assertion it is suf-
ficient to show that the restriction maps Hs (C*(X,XA)) —> Hs (C*{Xz,Xa))
are zero for s < k + 2 and (Z, -4) finite.
Fix Z. We first extend it to a larger finite subcomplex (Z[1],A) C (Y,A) so
that the inclusion ? : {?,A) —> (Z[l],.4) satisfies ??(?) = 0, s < k + 2. (This
implies ??(?) = 0, s < k + 2). Indeed the Cellular chain models theorem 4.18
shows that Ht(Z,A) has a finite basis. Let ui,...,% be cycles representing
the basis elements of degree < A: + 2. The hypothesis H-k+-(Y,A) = 0 implies
H<k+2(Y,A) = 0; hence uA = db\ in C*(Y,A). By compactness 6? G C*(Z\,A)
?
for some finite subcomplex (??,?) D (Z,A). Put Z[l] = \J Zx.
?=?
Finally, iterate this construction to give an increasing sequence (Z,A) C
(Z[l], A) C · · · C {Z[?], A) c · · · of finite subcomplexes such that the restriction
maps Hs (Z[?\, A) —> Hs (Z{? - 1], .4) are zero for s < k + 2.
For any subcomplex (?, A) C (Y, -4) recall that
(C*(?, .4) ® W, d) = C*(T,A) ® (C*(Y)®W,d).
In particular, suppose ? G C-m (? [1],.4) ®W is a cocycle of degrees < A:+2. We
remark that (C*(r?) ® id) ? is cohomologous to a cocycle in C-m+1(Z, A) ®W.
Indeed, writing ? = ?™ + $>m+i + ¦ ¦¦ with ?^ G C" (Z[l],.4) <g> W, and recalling
that d : Wj —> C*(—) ® W<j, we deduce from ?? = 0 that (d ® ??/)??? = 0.
Thus ?,? = ??? ® wa with daQ = 0. Since W is concentrated in non-negative
degrees m < s < A: + 2. Thus ?*(?)[??] = 0 and ?*(?)?? = dba. Evidently
{?*{?) ® id) ? - d (Eba ® wQ) G C^m+I (Z, A) ® W.
Put (?,?) = (Z[fc + 3],A) and let ? : (?, A) —> (?,/I) be the inclusion.
If ? G C* (?, ?) ® PF is a cocycle of degree s < A: + 2, it follows now that
{?*(?) ® id) ? is cohomologous to a cocycle in C^h+2{Z, A)®W. Since s < A;+2
and W = {W*}^, this cocycle is zero; i.e. Hs (?*(?) ® id) = 0, s < A; + 2.
Consider the commutative diagram
H'(XZ,XA) i H'(XT,XA)
|Hs(mr..4)
Hs {C*{Z,A) ® IF) < iis (C*(r.^
Homotopy Theory 83
The vertical arrows are isomorphisms (Case 3) for s < k+2. Thus Hs(Xt, Xa) —>
Hs{Xz,X\) = 0, s < k + 2, and so, a fortiori, is the composite HS(X,XA) —>
We now prove the proposition in general. Let (C,, d) denote the cellular chain
complex (§4(e)) for Y: Cn is free on a basis va corresponding to the n-cells
{e™}aeTn °f Y ¦ Since J: is a field we may choose subsets fCn C Jn C Tn such
that
Cn = (kero)n ? ? kva = (Imo)n © ? ???.
qgac„ oejn
Let yn be the ?-skeleton of the relative CW complex (Y:A), and define a se-
quence of subcomplexes ¦ ¦ · (S(n),A) C (T(n),A) C (S(n + 1), .4) C · · ¦ by
'S(n) = yn_x U ( U el ) and T(n) = rn_x U ( |J ena ) .
Using the Cellular chain models theorem 4.18 we see that
H*(T(n),S(n))=Hn(Y,A),
H*(S(n + l),T(n)) = 0
and
Hi{Y:S{k + 4)) = 0, i<k + 3.
By Cases 2 and 4, the morphisms mr(n)iS(n), ??^^^??,) and myiSn+4) are
all (k + 2)-regular. Since 5A) = .4 (because (Y,A) has no 1-cells or 0-cells) the
same argument as in Case 3 shows ???,? is {k + 2)-regular. ?
proof of Theorem 7.5: Choose a weak homotopy equivalence ? : (Z,zq) —>
(Y,yo) from a CW complex ? (Theorem 1.4). The construction in the proof of
1.4 shows that ? may be chosen with a single 0-cell, zq, no 1-cells and with all
cells attached by based maps. Let ? : Xz —> X be the pullback map. It, too;
must be a weak homotopy equivalence. Thus in the commutative diagram (cf.
G.3))
C*(Xz) < C {?) C*(X)
C*{Z) ®C.(Y] MY < C*(Y) ®c-(Y) My ,
both my and the horizontal arrows are quasi-isomorphisms; hence ?? ? is a quasi-
isomorphism too. Since H*(Z) S H*(Y), the pullback fibration satisfies the
hypotheses of 7.5. Thus since mz — my (cf. 7.7) it is sufficient to prove the
theorem for mz : C*(Z) <8>c-(y) My —> C*(XZ) and mz; i.e. we may assume
Y is a CW complex as described above.
Put H = H*(F;k) and let ? : (H,0) —> C*(F) be a (not necessarily mul-
tiplicative) morphism of cochain complexes satisfying ?{?) = id. We con-
struct now a C*(y)-semifree module (C*(Y) ®H,d) and a quasi-isomorphism
84 7 Semifree cochain models of a fibration
?? : (C*(Y)®H,d) —> C*{X) of C*(y)-modules such that
d:Hn ^C*(Y)®H<n, all ? and ?? = ? : (H,0) -^ C*(F).
Note that the first condition will imply that (C*(Y) ® H,d) is semifree.
Suppose d and ?? are constructed in C*(Y) ® H<n. Consider the row-exact
commutative diagram
0 ^ c*(X, F) ^ C*(X) *- C*{F) *- 0
0 ^(C*(Y,y0) ®H<n,d) ^ (C*(Y)®H<n,d) ^(H<n,0) ^0.
Since ?? = ? is (? — l)-regular by construction, Proposition 7.8 asserts that
??,?? is (n + l)-regular. Let {7^} be a basis of Hn and lift the cocycies 6jk
to elements xk G Cn(X). Then dxk is a cocycle in Cn+1(X,F) and so dxk =
?Y,yo(uk) +dvk for some cocycle uk G C(Y,y0) ®H<n and some vk G Cn(X,F).
Extend d and ?? by setting d^k = uk and ??{??) = Xk — vk. In this way
(C*(Y) ®H,d) -^ C*{X) is constructed.
Since ?? is the quasi-isomorphism ?, Proposition 7.8 asserts that ??^0 is a
quasi-isomorphism too. Hence, by the five lemma, so is ??.
Finally, consider the diagram
(C*(Y)®H,d)
Since ?? is semifree we may (Proposition 6.4 (ii)) find a C*(y)-module mor-
phism ? : ?? —> (C(Y) ® ii,d) and a C*(y)-linear map h : MY —> C*(X)
such that
— my = dh + hd.
In particular ?(??) ? ?{?) = ?(???) and 1/ is a quasi-isomorphism.
It follows by Proposition 6.7 (ii) that u factors to give a quasi-isomorphism
? : Jfe <g>c-(Y) MY —> J: ®c.(y) (C*(F) ® ii,d). Similarly, since ? is C*(Y)-
linear, a linear map h : h ®c(Y) ?? —> C*(F) is defined by the commutative
diagram
C*(F)
h ®C(Y) ?? < My
Homotopy Theory 85
It is immediate from this construction that ?,?? — ??,? = dh + hd. Hence
?(???) = ?(??) ??(?>) is an isomorphism. ?
We finish this section by extending Theorem 7.5 to a somewhat more general
setting. We have worked till now with the square
c'(j)
k < C*(Y).
Now suppose more generally that
-fi»
??? . ?
is any commutative square of cochain algebra morphisms. A quasi-isomorphism
V -=> V(l) from ? to a second such square D(l) consists of cochain algebra
quasi-isomorphisms
?:?-=>?A), 7:?-=>?A) and a : A-=> A(l)
that define a commutative cube connecting T> to T>A). In particular, we shall
say that the squares T>c and T> above are weakly equivalent if they are connected
by a finite chain of quasi-isomorphisms of the form
V ^ V{1) A- X>B) ^ ¦¦¦^¦Vc .
Now consider the square V. Left multiplication by ?F), b G ? makes ? into
a left B-module for which (Proposition 6.6) there will be a semifree resolution
m ? '· Mb ^> E. From this we may, as in G.4), define a morphism m b of
complexes by the commutative diagram
.4 <-$— ?
*jme G.9)
k ®b Mb < ;— ? (8>b Mb .
Theorem 7.10 Suppose k is a field, ? : X —> Y is a fibration with Y simply
connected and that one of the graded Ik-vector spaces H*(Y), H'(F) has finite
type. If T> is a square weakly equivalent to Vc and if tub · Mb -^ ? is a
B-semifree resolution then
rnB · -& ®b Mb
? quasi-isomorphism.
86 7 Semifree cochain models of a fibration
proof: Suppose given a quasi-isomorphism V —> V{\) as above. Then we have
the commutative diagram
?A)
? ®B MB ~ ) -B(l)
i?®d
which identifies m as a quasi-isomorphism. From this we deduce that m =
a o ifiB · & <S>b MB —> A(l).
On the other hand, suppose m(l) : M(l) -=> E(l) is any B(l)-semifree res-
olution. Then, exactly as in the last part of the proof of Theorem 7.5, we
can find a quasi-isomorphism ? : M(l) —> B(l) ®b Mb of B(l) modules such
that m ? ? is ??(l)-homotopic to m(l). Since ? is a quasi-isomorphism so is
? : ]k ®b(i) M(l) —> M ®b Mb· Moreover, exactly as at the end of the proof of
Theorem 7.5, H{M) ? H(u) = H(m(l)). Altogether we conclude that
tiib is a quasi-isomorphism <=$¦ misa quasi-isomorphism
-?=> ??A) is a quasi-isomorphism.
Consider the chain connecting V to T>c, and recall that the semifree model
for T>c is just ??? : ?? —> C*(X). Thus the argument above, repeated along
the chain, shows that tub is a quasi-isomorphism if and only if ??? is. But ??
is a quasi-isomorphism by Theorem 7.5. ?
Exercises
1. Prove the converse of Theorem 7.5.
2. Let ? : X -> Y be a fibration with simply connected base. Assume Ik is
a field and that H*{F;Ik) has finite type. Let (R,d) -> C*(Y,Ik) be a quasi-
isomorphism of cochain algebras. Prove that the fibration ? admits a minimal
semifree resolution (§ 6-exercise 7) of the form (#<8> ?, d) with ? — H*(F; Ik)
and d(Hk) C R®H<k. Write, for any ? G ?, ?? = ??? + ... + ??? + ... with
??? G {Rn ® ?). Prove that this sum is finite and if ^? = ... = ??? = 0
that ??+1? is a cocycle in (R® H,d). A class ? is transgressive if there exists
? such that ??? = ... = ??? = 0 that ???+1? ? 0. Prove that this definition
does not depend on the choice of the minimal resolution and that the set ?
of transgressive classes is a vector space, so that ? ?-» [dn+1 ?] defines a linear
map Tn 4 Hn+1(Y). A fibration is totally transgressive if H*(F,Ik) = T.
Characterize the minimal resolutions of totally transgressive fibrations.
3. In exercise 2, assume F = Sn. Prove that the fibration admits a minimal
semifree resolution of the form (R®Ax/(x7),d) with dx G Rn+1. The cohomology
class ? = [dx] G Hn+1{X;Ik) is called the Euler class of the fibration. If ? is
odd, prove that we have a short exact sequence 0 -> (R, d) -> {R® Ax, d)-
Homotopy Theory 87
Ikx, d (8> id) —» 0 with associated long exact sequence ( Gysin exact sequence)
4. In exercise 2, assume Y = Sn. Prove that this fibration admits a resolution
of the form (?6/F),0) -> (Ab/(b'2) ® H,d) 4 (tf,0) with ??? = b ® ?(?), ? G
ff, deg b = ?. Deduce that there is a short exact sequence 0 —» (J7c6 <8> if, 0) —»
(/\b/(b2) <8> H, 0) -> (if, 0) -> 0 with associated long exact sequence ( Wang exact
sequence) ... -> iP(F) -> ^+?(?) ^ Hi+n(F) -> if'+1(F) -> ....
5. Prove that the homotopy fibre of the canonical projection S2 VS1 -> 51 is an
infinite bouquet of 2 dimensional spheres. Does the conclusion of theorem 7.5
hold?
6. Let F ? ? ? ? be a fibration as in exercise 2. Assuming that ??;(?) is an
isomorphism for i < n, deduce that H{{p) is an isomorphism for i < n.
7. Let (X,A) be a pointed pair. Construct, for each ? > 1, a natural map
h(.x,A) '¦ ??(?,?,??) —? Hn(X,A;Z) such that (hA,h.x,h(xtA)) maps the ho-
motopy long exact sequence into the homology long exact sequence of the pair
(X, A). Deduce Whitehead's theorem from exercise 6: Let / : X —» Y be a
continuous map with .Y and Y path connected. If (??^./) <gi ifc is an isomorphism
for k < ? and is surjective for k = ? then Hk{f; Ik) is an isomorphism for k < ?
and is surjective for k = n.
8 Semifree chain models of a G-fibration
As usual, we work over an arbitrary commutative ground ring, h. For simplicity,
we denote C»{—\k) simply by C*(—) and H^(—\k) simply by Ht(—).
In §2(b) we defined the action of a topological monoid, G and the notion
of a G-fibration. Here we show how multiplication in G makes C*(G) into a
chain algebra, and how an action of G in a space ? makes Ct{P) into a C*{G)~
module. Then, in the case of G-fibrations ? A I we show (Theorem 8.3) that:
if (M,d) -?» C*(P) is a C«(G)-semifree resolution then there is an induced
quasi-isomorphism (M,d) ®c.(G) ¦& —> C,(X).
Notice that this is the exact analogue of Theorem 7.5.
Using Theorem 8.3 we then give a geometric application of the isomorphism
theorems of §6. Finally we apply this to provide an elementary proof of the
Whitehead-Serre theorem (Theorem 8.6):
// k is a subring of Q and if ? : X -> Y is a continuous map between
simply connected spaces then ?\»{?) ®z h is an isomorphism if and
only H*(ip;]k) is an isomorphism.
This section is divided into the following topics:
(a) The chain algebra of a topological monoid.
(b) Semifree chain models.
(c) The quasi-isomorphism theorem.
(d) The Whitehead-Serre theorem.
(a) The chain algebra of a topological monoid.
Suppose G is a topological monoid. To make Cm(G) into a chain algebra
we use the Eilenberg-Zilber chain equivalences introduced in §4(b). If ? is the
multiplication in G then we assign to C»(G) the multiplication
C,{G)®C,{G)
It is associative because ? ? and ? are. The identity 1 € C»{G) is the 0-simplex
at the identity e € G. By C*(G)-module we shall mean a module (M, d) over the
chain algebra, C*(G).
The constant map G —> pt may be regarded as a map of monoids, and so the
augmentation
e:Cm(G;k) —> Jfc (8.1)
is a dga morphism. In particular it makes Jk into a C«(G; Jt)-module.
In the same way a right action ?? : ? ? G —> ? induces the morphism
CJun) ? ?? : C.(P;k) ® C*{G;k) —> C.(F;Jfc), which makes C*(P;k) into
Homotopy Theory 89
a right C»(G; Jc)-module. In the case of a G-Serre fibration ? : ? —> X the
commutative diagram
? ? {pt} ——> ?"
translates (cf. D.5)) to the algebraic formula
C.(p)(u · a) = e(a)C.(p)u, u € C.(P), a € C,(G).
Thus a morphism, m : M —> C*(P), of right C« (G)-modules induces a unique
commutative diagram
(?), ?? = x®c.(G) 1, a: € M . (8.2)
of chain complexes. When m = id : C*(P) —> C*(P) we write ? = C»(p) :
(G)^ —> C»(X). Otherwise we will usually denote ? by m : M®c.(G)
(b) Semifree chain models.
In general, C,(p) is not a quasi-isomorphism. However, if we replace C»{P)
by a C« (G)-semifree resolution, we have
Theorem 8.3 Suppose ? -^ X is a G-Serre fibration and m : M -=> C»(P)
is a C»(G)-semifree resolution. Then
m: M ®c.{G) * —> C*(X)
is ? quasi-isomorphism.
The main step in the proof of Theorem 8.3 is the construction of a certain
'geometric' C"*(G)-semifree resolution of C*(P) when X is a CW complex. This
is a simple generalization of the cellular chain models of §4(e). We first carry
out this construction, and then prove Theorem 8.3.
Thus we suppose X is a CW complex with ?-skeleton Xn. Recall from §4(e)
that a cellular chain model for X is a quasi-isomorphism q : (C, d) -%¦ C*(X) that
restricts to quasi-isomorphisms qn : (C<n,<9) -^» C*(Xn), ? > 0. In particular
In induces an isomorphism Cn -^» ??(??,??_?), and this identifies Cn as a
free Jfc-module on a basis {cQ} corresponding to the ?-cells of X. Moreover d is
identified with a certain connecting homomorphism.
Denote by ? : Pn —> Xn the G-fibration obtained by restricting ? to Pn =
90 8 Semifree chain models of a G'-fibration
Definition A cellular chain model for the G-fibration ? : ? —> X is a quasi-
isomorphism of right C,(G)-modules of the form
m:(C®C.(G),d) 4C,(P),
restricting to quasi isomorphisms m(n) : (C<n ® C*(G), d) -^> C*(Pn), and such
that
m : (C,d) = (C®C.(G),d) ®C.(G) * —> C.(A')
is a cellular chain model for X.
Remark Since C,{G) is concentrated in non-negative degrees necessarily d :
Cn ® 1 —> C<n <8>O,(G). This exhibits m : (C®C.(G),cQ —> C.(F) as a
C,(G)-semifree resolution.
Proposition 8.4 Every G-Serre fibration whose base is a CW complex has a
cellular chain model.
proof: Denote the fibration by ? : ? —> X and adopt the notation above, so
that the ?-cells of X form a basis, cQ, of Hn(Xn,Xn-i). We show first that
Hm(Pn,Pn-i) is a free H«(G)-module on a basis aQ € Hn(Pn, Pn-i) satisfying
H,(p)aQ = cQ. Write Xn = Xn-i U fljes) and let / : fIJ^2,IJ^2
(Xn,Xn-i) be the characteristic map. Write {D,S) = ( U-D^US^ ) and
\ Q Q /
use / to pull the fibration ? —? ? back to the G-fibration
PD = DxxP^ D, (y,z)-g = (y,zg), (y,z) € F/j, g € G.
Let Ps be the restriction of PD to S, and let ? : (Fd,Fs) —> (Fn,Fn_i) be the
pullback map. We first observe that H,(f) and ? „(?) are isomorphisms.
Indeed, if oQ is the centre of DJ put ? = D - \\{oa}, U = Xn - ]J{oQ},
a a
Po = p?(O) and Pc/ = p-^C/). The inclusions S^—>O and ?'?_? <=—>*/ are
homotopy equivalences, and hence P5 —>¦ Po and Pn-i c—> Pu are weak homo-
topy equivalences (Proposition 2.3). Exactly as in §4(e) it follows by excision
that H,(f) and ?*(?) are isomorphisms.
Choose a contracting homotopy Dxl—> D from the map ]\ D%—> \J {o?}
to the identity. Choose points zQ € p~jj{oa) and lift this homotopy to a map
? : D ? I —> PD, starting at the map ]} #2 —> LI {^2}- Denote ii(-, 1) by
? : D —> PD. Then pD ? ? — id. Let D x G —> D be the trivial G-fibration
(with action (x,g) ¦ gx — {x,ggi)) and define
(D,S)xG * >- (PD,PS)
(D,S)
Homotopy Theory 91
by ip(x,g) = ?(?) ¦ g. By the very definition of G-Serre fibrations ? restricts to
weak homotopy equivalences in the fibres. Hence (Proposition 2.3) ? restricts
to a weak homotopy equivalences D ? G —> Pp and S x G —> Ps- It follows
that ? „(?) is an isomorphism.
Because Hm{D,S) = Hn(D,S) is a free module (on the ?-cells of X), there is
a chain equivalence Hn(D,S) -^ C*{D,S). Thus the composite
Hn(D,S)®Cm(G)
^ C((D,S)xG)
defines an isomorphism ?(?) : Hn(D,S) <g> i/,(G) —> i/»(Pn,Pn_i). The asso-
ciativity of the Eilenberg-Zilber map implies that ^Z above is a morphism of
C»(G)-modules. Hence ?(?) is an isomorphism of H*{G) modules.
Let aa € Hn(Pn,Pn-i) be the image of X(n)(cQ <8> 1). Then Hn(Pn,Pn-i) is
a free right //„(G)-module with basis {aa}, and Hm(p)aQ = cQ.
We now construct the semifree resolution m : (C'<8> C,(G),d) —>¦ C*(P) by an
inductive process. Assume the quasi-isomorphism m(n — 1) : (C<n_i ® C*(G),d)
-^> C»(Pn_i) is defined. Let 6Q € Cn(Pn) be an element that projects to a
cycle zQ € Cn(Pn)-Pn-i) representing aa. Then d6Q is a cycle in C,(Pn-i).
Choose a cycle ua € C<n_i ® C*(G) and an element wQ € Cn(i?n-i) so that
m(n — \)(ua) = d6Q + dwQ. (Recall H(m(n — 1)) is an isomorphism.) Extend d
and m(n — 1) to C<n <8> C»{G) by setting d(cQ ® 1) = «Q and m(n)(cQ ® 1) =
bQ + wa. The map C<n/C<n <S> C,(G) —> C*(P„,P„_i) induced from m(n)
produces, in homology, the morphism I 0iccQ 1 <S> H,{G) —> H,(Pn,Pn-i) of
V a J
#»(G)-modules given by cQ i-» aa. This is the isomorphism ?(?). Thus by
the Five lemma 3.1, m(n) is a quasi-isomorphism. This completes the inductive
construction of m : (C <8> C,{G),d) —>¦ C*(P). Since any singular chain in ?
lives in a compact subspace it must, in particular, be contained in some Pn. It
follows from this, and the fact that each m(n) is a quasi-isomorphism, that m is
a quasi-isomorphism too.
Finally, by construction, m(cQ <8> 1) = 6Q + wQ projects to a cycle 2Q €
Cr»(Pn,pn_1) such that C,(p)zQ represents cQ. This is precisely the condition
that m : (C, d) —> C.(X) be a cellular chain model. D
proof of Theorem 8.3: We are given a G-fibration ? A X and a C,{G)-
semifree resolution m : M A C»(P). Let 5 : Y —> .Y be a weak homotopy
92 8 Semifree chain models of a G—fibration
equivalence from a CW complex Y (Theorem 1.4) and form the pullback
Y*xp * . ?
?
? is a map of G-fibrations and a weak homotopy equivalence (Proposition 2.3).
Let ? : (C ® Ct(G),d) -^» C*{Y x.v P) be a cellular chain model for our G-
fibration; its existence is guaranteed by Proposition 8.4. Recall (diagram (8.2))
that ? factors to define
? : (C ® C,
Since (C <8> C»(G),d) is C,(G)-semifree we may, in the diagram
M
(C®C.(G),d) _?_^?(?),
lift Ct(ip) ? ? through m to obtain a morphism ? : (C 0 C,(G), d) —> M and a
C.(G)-linear map h : C ® C'.(G) —> C(P) such that mi/ - 0»{?)? = dh + hd
(apply Proposition 6.4 (ii)). Since m, C»{ip) and ? are quasi-isomorphisms so is
v. Thus (cf. Proposition 6.7 (ii))
is a quasi-isomorphism.
Finally, since h is C« (G)-linear we can form
h:[C®C,
Evidently fhv-C»{g)]i - dh + hd. Since i>, C*{g) and /x are quasi-isomorphisms,
so is m : M ®c,(G) & —> C»(X). ?
(c) The quasi-isomorphism theorem.
Suppose given
• a morphism a : G —> G' of topological monoids, and
Homotopy Theory 93
• a commutative square of continuous maps
where ? is a G-fibration, p' is a G'-fibration and ?(??) = ??(?)?(?), ? €
P,aeG.
Then C»{a) is a chain algebra morphism. Thus it makes any C«(G')-module
{eg. Ct(P')) into a C« (G)-module. Moreover Ct(<p) is a morphism of C*(G)-
modules:
C.M(u · *) = [C.{<p)u] ¦ [Cm(a)x], u € C.(P),x e C(G).
Theorem 8.5 W^ii/i ?/ie notation above, assume G is path connected and C(a)
is a quasi-isomorphism. Then
C*(<p) is a quasi-isomorphism <=? 0»{?) is a quasi-isomorphism.
proof: The proof of Proposition 6.6 shows that C.(P) and C.(P') admit C*(G)
and C»(G') semifree resolutions
m:(M,d) A C.(P) and m' : (M',d) -^ C.(P')
with M and M' concentrated in non-negative degrees. Since (M, d) is semifree
and m' is a quasi-isomorphism we can find
/ : (M, d) —> (M', d) and O : M —> C.(P')
such that / is a morphism of C«(G)-modules, O is C«(G)-linear and m'/ =
C,(ip)m + ?? + ?? (Proposition 6.4 (ii)).
Recall the notation of Theorem 6.12, which we wish to apply here, noting that
Hq (Ct(G)) = Ho (G.(G')) = Jk. Diagram (8.2) and its analogue for the fibration
? yield the following diagram of chain complex morphisms:
C.{X) > C.(X').
CM
This diagram may not commute. However, because ? is C«(G)-linear it factors
to give a linear map ? : M ®c.(G) k -+ C»{X') and m'(/®c.(Q) it) = C»{i>)m +
94
8 Semifree chain models of a G-fibration
?? + ??. Thus C*(tp) is a quasi-isomorphism if and only if / is and C*{ip) is a
quasi-isomorphism if and only if / ®c*(a) & is. Now apply Theorem 6.12. D
Theorem 8.5 has the following important corollary. Consider a fibre preserving
map between Serre fibrations ? and p':
X
f
X'
and let fy : Xy —> X'gy be the restriction of / to a map between fibres.
Corollary to Theorem 8.5 Assume Y and Y' are simply connected and X
and X' are path connected. If C+(Clg) is a quasi-isomorphism then
Cr(f) is a quasi-isomorphism <?=> C»(fy) is a quasi-isomorphism.
proof: The construction in §2(c) of the holonomy fibration is natural. Thus
there are commutative diagrams
X
PY fxPg ' X1 xY. FY'
and
X
f
X'
X XYPY
with ? and ?' weak homotopy equivalences. Since Y and Y' are simply connected,
UY and ?.?' are path connected. Now apply Theorem 8.5. to the first diagram.
D
(d) The Whitehead-Serre theorem.
Theorem 8.6 (Whitehead-Serre) Suppose Jk is a subring of Q and g : X ->
Y is a continuous map between simply connected spaces. Then the following
assertions are equivalent:
(i) TT*(g) <8>z -& is an isomorphism,
(ii) Ht[g\]k) is an isomorphism.
(Hi) H*(Qg;]k) is an isomorphism.
Homotopy Theory 95
Remark Here ?, (g) ®z k denotes the homomorphism ?* (g) ®? idjk : ?» (?') <8>?
k —> irt(Y)®zk. We emphasize the role of k by using the full notation C« (—;k)
and #«(—; k). When k = ? the theorem reduces to Whitehead's original result:
g is a weak homotopy equivalence if and only if Ht(g; Z) is an isomorphism.
Lemma 8.7 Suppose k C Q, X is an (r — l)-connected space and either r > 2
or r = 1 and tti(X) is abelian. Then the Hurewicz homomorphism defines an
isomorphism
*r(X)®Zk = Hr(X;k).
proof: By Theorem 4.19 the Hurewicz homomorphism is an isomorphism nr(X)
-=> Hr(X;Z). Thus it defines an isomorphism nr(X) <S>z ¦& -^> Hr(X;Z) ®z Ik.
On the other hand the identification C*(.,Y;Z)<g>z.& = C»(X;1;) defines a map
ii,(.Y;Z) <8>z & -> H,(X;]k). Because Je C Q the operation -®zA preserves
exactness, and it follows that this map too is an isomorphism. ?
We shall make frequent use of Eilenberg-MacLane spaces (§4(f)). (The reader
may wish to review §4(f), since we shall often use there properties established
without explicit reference.) Finally, we shall also rely on the
Proposition 8.8 Suppose h C Q and X is an Eilenberg-MacLane space of
type (?r, ?), ? > 1, with ? abelian. Then
?®?* = 0 «*¦ H,{X;k) = H,{pt;k).
proof: Since ir®zl: = Hn(X; k) we have only to prove that if ? <S>z k = 0
then Ht(X;k) = Ht(pt:k).
Case 1: ? = 1 and it is cyclic.
Since ? <g>z A = 0, there is a short exact sequence
where ? is a multiplication by an integer k invertible in k. Let / : K(Z,2)
?(?,2) be a map such that ???(/) = C and convert / to a fibration
The long exact homotopy sequence identifies F as an Eilenberg-MacLane space
of type (Z5 2) and identifies n2(i) with the homomorphism ? : ? -> ?.
Now consider the commutative square
UE
??, 2)
96 8 Semifree chain models of a G-fibration
as a map of QF-fibrations, where the right hand side is the loop space fibration
described in §2(b). The long exact homotopy sequences for the path space fi-
brations identify QF and ?.? as Eilenberg-MacLane spaces of type (Z,l) and
identify ??^??) with ?. Thus Hi(O.i; k) = ???(??) ®? k is an isomorphism.
Moreover, because the universal cover, E, of S1 is contractible it follows that
S1 is an Eilenberg-MacLane space of type (Z, 1). Since all Eilenberg-MacLane
spaces of the same type have the same homology, Hi(Q.E;k) = Hi(O.F;lc) =
0,i > 2, and so Ht(Cli,]k) is an isomorphism.
We can therefore apply Theorem 8.5 to the map of OF-fibrations above to
conclude that ?»{??(??,2)\?) - H*{pt\k). But ??{?,2) is an Eilenberg-
MacLane space of type (?, 1) and hence has the weak homotopy type of X,
whence H.(X;k) = H«{pt\k).
Case 2: ? is cyclic.
We induct on n, the case ? = 1 having been established in Case 1. Consider
as a map from an {e}-fibration to the ?.?-path space fibration on X; {e}
denoting the 1-point monoid. By the induction hypothesis, H*({e};k) -^
Ht(QX,k), since CtX is an Eilenberg-MacLane space of type (??,? — 1). Ap-
ply Theorem 8.5 to conclude that H,(pt;h) -=> H,(X;h).
Case 3: The general case.
It is easy to modify the construction in §4(f) of cellular Eilenberg-MacLane
spaces to construct a ?{?, ?) that is the union of sub CW complexes ?(??, ?) as
? a runs through the finitely generated subgroups of ?, and such that K(nQ,n) —>
?"(??, ?) induces the inclusion ?? —? ?. We may also assume that for any ??, ao
there is a ? such that ?(???,?) D ?(???,?), i — 1,2. Since — ®z h is exact,
each ?? ®z & = 0. If we can show that each ?,(?(??; ?); fc) ~ H,(pt; Jfc) it will
automatically follow (eg via cellular chains) that ?*(?(?,?); Je) = Ht(pt;Jk).
Since X has the weak homotopy type of K(n,n) this will complete the proof.
We may therefore as well assume that X is one of the ?(??,?); i.e., that ?
is finitely generated. Then ? is the finite direct sum ?? ? ... ? Fm of cyclic
groups and each ?* <g>z h = 0. Thus X has the weak homotopy type of the
product Y\^LiK(Ti,n), and, by Case 2, there are chain equivalences fc -=>
C» (K(Ti, n); Jk). Since the tensor product of chain equivalences is a chain equiv-
alence, the Eilenberg-Zilber chain equivalence gives
Homotopy Theory
ThusHt(X;k) = H,{pt-k).
97
D
proof of Theorem 8.6: Apply the Cellular models theorem 1.4 and the
Whitehead lifting lemma 1.5 to obtain a based homotopy commutative diagram
X'
hx
X
Y'
Y
in which X' and Y' are CW complexes and hx and hy are weak homotopy
equivalences. Since ?/?? and Clhy are also weak homotopy equivalences it is
enough to prove the theorem for g': i.e., we may assume .Y and Y are CW
complexes, which we now do.
Our first step is to reduce to the case that T\2{g) is surjective. Let ? =
Ko(Y)/\miT2{g) and let ? = K(T,2). Use Proposition 4.20 to find a continuous
map q : Y —> ? such that ^(g) is the quotient homomorphism. Thus T\i{qg) — 0
and so qg is based homotopic to the constant map.
In §2(c) we showed how to convert q to a fibration q via the commutative
diagram
Y a ? ?? ??
in which ? is a homotopy equivalence. Since q Xg is homotopic to the constant
map and q is a fibration we may lift the homotopy to a homotopy Xg ~ g\ with
5i mapping X into the fibre ? ?? PK- By the long exact homotopy sequence,
"E1) is surjective, and we claim it is sufficient to prove the theorem for g\.
In fact the claim follows from the assertion that if i : ? ?? PK —> ? ?? ? ?
is the inclusion then ??*(?) ® Jk, H,(i;L·) and Ht(Cli,k) are all isomorphisms,
which we see as follows. The Hurewicz theorem 4.19 identifies H2(g) = ^2E) =
Ti(Qg) = Hi(Clg). Hence under any of the three assertions of the theorem,
"E) ®z Jk is an· isomorphism. Since — ®z k is exact ? ®? h = 0, and so
?*(?) ®z Jk is an isomorphism.
Moreover Proposition 8.8 asserts that ?»(?;3? = Jk = Ht(QK,k). Apply
the Corollary to Theorem 8.5 to the fibre preserving map
Y xKPK
Y xK MK
pt
?
98
8 Semifree chain models of a G-fibration
to conclude that Ht(i;k) is an isomorphism. On the other hand, 7r2(c) is sur-
jective since 7r2(c) is. Thus ??(?) is injective and ? ?? PK is simply con-
nected. Looping the diagram above gives a map of ?,(? ? ? Pii)-fibrations.
Since ?(?" ?? ??) is path connected Theorem 8.5 can be applied to deduce
that H,(Qi;k) is an isomorphism.
In view of this discussion we may and do henceforth assume that iio(g) is
surjective. We first establish that assertions (i) and (ii) are equivalent.
Convert g into the fibration
?: ? xy MY —>Y
as described in §2(c). The fibre of ? is A' x y PY and the homotopy equivalence
? -=> ? ?? MY identifies g with p. Thus the long exact homotopy sequence
shows that ? ?? PY is simply connected. Moreover, since — <g>z k is exact,
TTr,(g) ® k is an isomorphism
On the other hand (cf. §2(c)),
X xy PY
?,(? ?? PY) ® it = 0.
PY
X 5 ~Y
is a morphism of Qy-fibrations. Thus Theorem 8.5 asserts that
Hm(g,k) is an isomorphism 4=> H,(X ? ? PY: k) = H,(jpt;k),
Let F —> X Xy PY be a cellular model. We have only to show that
7T,(F)<8)ZJt = 0 <=> H*(F;k) = H.(pt;k). (8.9)
Define a sequence {Fr}r>i of r-connected CW complexes, beginning with F\ =
F, as follows. Given Fr; put Kr+\ = K(nr+i(Fr), r + 1) and choose a
continuous map
so that 7??+?@?) is the identity isomorphism. Then let Fr+1 be a cellular model
for the homotopy fibre Fr XKr+l PKr+\ of 6r.
As described in §2(c) we have a morphism of Qiir+1-fibrations,
Fr XKr+1
????
(8.10)
Fr
???
Homotopy Theory 99
Moreover, the long exact homotopy sequence has the form
... ? nn(Fr xKr+1 PKr+l) ^H *n(Fr) ^H ??(??+1) ? ....
It follows that Fr x-Kr+l PKr+\ is indeed (r + l)-connected and that ??(??) is
an isomorphism for ? > r + 2. Thus we have isomorphisms
7rr+1(Fr) A 7rr+1(Fr_i) A ... A 7rr+1(F)„ r > 1.
Suppose now that H»(F;L·) = H,(pt;]k). We show by induction on r that
H*(Fr;]fc) = H,{pt;L·), noting that this is true by hypothesis for r = 1. If this
holds for some r then ?rr+1(Fr) ®zi = Hr+i{Fr\L·) = 0 and so Proposition
8.8 asserts that H*{Kr+i\]k) = H,(pt;]k). Hence ??(??;1?) is an isomorphism.
Now Theorem 8.5, applied to the diagram (8.10), asserts that H,(Or,]k) is an
isomorphism, whence
H.(Fr+1;k) S H.(FrxKr+lPKr+i) as H.(PKr+1;k) = H.(pt;k).
Since this is true for every r, the homotopy group isomorphisms above show that
7rr+i(F) ®z k = nr+i(Fr) ®z k = Hr+1(Fr]]k) = 0, r > 1,
as desired.
Conversely, suppose ttj(F) ®z J: = 0, i > 2. Then the homotopy group
isomorphisms above show that ni(Fr) <S>z & = 0 for all i > 2 and r > 1. In
particular, 7rr+1(Fr ?? Jk) = 0. On the other hand, if r > 1 then QKr+i is
an Eilenberg-MacLane space of type Grr+1(F),r + 1). Hence (Proposition 8.8)
H*(QKr+i;k) = H.(pt;k) for r > 1. Consider the diagram
Fr xKr+1 PKr+x -U. Fr xKr+1 PKr+l
id.
Fr *Kr+l PKr+l Fr
as a morphism from an {e}-fibration to an Qiir+1-fibration, {e} denoting the 1-
point monoid. Apply Theorem 8.5 to conclude that H»(pr; 3c) is an isomorphism,
? > 1. This gives a sequence of isomorphisms
A H.(Fr+l;k) A H.(Fr;k) A - · · A ff.(Fi; Jfc) = H.(F;k).
Since Fr is r-connected it follows that H.(F;k) = H,{pt;k).
This completes the proof that assertions (i) and (ii) are equivalent. To see
that (ii) and (iii) are equivalent we recall that we may assume ito(g) is surjective
100 8 Semifree chain models of a G-fibration
and we use the notation and constructions above. Theorem 8.5, applied to
PX -^- PY
? 9 X
implies that if H.(Qg;k) is an isomorphism so is Ht(g;k).
Conversely, suppose Ht(g;k) is an isomorphism. Let Fg = X xy PY denote
the homotopy fibre of g. Then, as shown above, Ht (Fg; k) = Jk and ?, (g) ®z k
is an isomorphism.
On the other hand, in §2(c) we constructed an ??-fibration q : P'X xxFg —>·
Fg such that the fibre inclusion j : ?.? —> P'X xxFs was identified with Qg
by a homotopy equivalence. Thus tt«(j) ®? k is an isomorphism by hypothesis
and we have only to show that H» (j; k) is an isomorphism.
But the commutative diagram
QX 3- P'X *xFg
{pt}
is a map of ??-fibrations and the map {pt} —> Fg induces an isomorphism in
homology. Now Theorem 8.5 asserts that H,(j;k) is an isomorphism too.
D
Exercises
1. Let Jfc[G] = {%2geG^g g. Afl € Ik}, where \g takes the value zero except
on a finite number of elements of G, be the group ring of a group G. Construct
a quasi-isomorphism (Jk[G],0) 4 C*(G;Ik)). If ? -> X is a G-fibration (for
instance a finite covering) prove that there exists a quasi-isomorphism of Ik[G]-
modules Ik[G] ® C,{X\Ik) 4 C.(P;Ik).
2. Let ? € CiiS1;^) be defined by o{t) = e2i7rt. Prove that ? is a cycle such
that ?2 = 0. Construct a quasi-isomorphism of algebras (Ax, 0) —>
3. Let ? be the torus S1 ? S1 ? ... ? S1 (? times). Deduce from exercise 2 a
quasi-isomorphism of algebras ? : (?(«?, ?2,..., xn), 0) -> G, (T). Assume that T
acts from the right on a topological space X. Prove that C*(X; Ik) inherits, via
?, a left (?(??,?2, -.,??), 0)-module structure.
4. Let F -> X -> Y be a fibration with Y 1-connected and consider the associ-
ated ny-fibration UY -> F -> X. Prove that if H,(F;Ik) is a free Ht(UY;Ik)-
module, then Ht{X;Ik) = Ik ®?.(?^;?·) H.(F;Ik).
Homotopy Theory 101
5. Prove that the spaces ?' = UPm ? 5" and Y = Sm x RPn have the same
homotopy groups but not the same homology groups. Does this contradict the
Whitehead theorem?
6. Let ? -> X be a G-fibration with ?' 1-connected. Prove that if (T(V),d) 4
C, (ClG) is a quasi-isomorphism of chain algebras then there is a weak equivalence
C*{X) 4 HomT(v)(T(F) ® M, Ik), and describe the semifree module TV <g> M.
9 P-local and rational spaces
Fix a set ? of prime numbers and let 1 be the set of integers k relatively prime
to the elements of P. An abelian group ? is V-local if multiplication by k in ? is
an isomorphism for all k € ?. The rational numbers m/k, k € ?, are a subring
Jk C Q, so ? is P-local if and only if it is a A-module. By convention, when
? = ? we take it = Q — in this case ? is a rational vector space. Throughout
this section our ground ring Jk will be the subring associated in this way with the
set of primes P.
If 0 -> ?' -> ? -> ??" -> 0 is a short exact sequence of abelian groups, then it'
and ?" are P-local if and only if ? is P-local. For any abelian group ?, ? ®?&
is P-local. Moreover, if ? is P-local then ? -> ? ®% k is an isomorphism. For
general ? this homomorphism
? —> ? ®z Jk , 7 ?—» 7 ® 1
is called its V-localization.
The idea of P-local spaces and localization of spaces, introduced by Sullivan
in [143], is a topological analogue. As will be shown in Theorem 9.3, if X is a
simply connected space then
tt.CX") is P-local <=*· Ht(X,pt;Z) is P-local.
This motivates the
Definition A simply connected space X is a V-local space if it satisfies these
equivalent conditions. When L· = Q (i.e., V = ?) ? is called a rational space.
This section is organized into the topics:
(a) P-local spaces.
(b) Localization.
(c) Rational homotopy type.
(a) V—local spaces.
Before turning to the proof of Theorem 9.3, we introduce an important class
of P-local spaces: the (relative) CW-p-complexes , beginning with the classical
motivating example: the infinite telescope Sj,.
Let hi, ko,... be the positive integers relatively prime to ? (i.e.; the denomi-
nators of Jk). Put
Homotopy Theory 103
where DJ+1 is attached by a map 5" -> Sf_x V S? representing [Sf^] - kj[S?].
For ? = 1 this is illustrated by the picture
? hi x k-2 x k3
in which the (j — l)st right hand circle is attached to the jth left hand circle
by the map e2"u h-» e2lzikit, and the bottom solid lines are identified to a single
point. (This explains the terminology: 'telescope'.)
The space S? is called the V-local ?-sphere and the space Dp'1 - S? ? I/S? ?
{0} is called the V-local (n + l)-disk. When Jk = Q these spaces are called the
rational ?-sphere Sq and the rational (n + l)-disk, D^+1. The inclusion of the
initial sphere 5J -> S? extends to Dn+1 -> ?>? and this inclusion
is called the natural inclusion of the initial disk and sphere. (This is the simplest
example of 'P-localization of topological spaces, defined and constructed later in
this section.)
Now we compute the homolog}' of Sj,, showing that
? i = 0
Jt i = n
0 otherwise
with the generator 1 G Hn(Sj,;Z) represented by the initial sphere [5J]. For
this, let X(r) C S? be the sub complex V 5f Uh ( JJ Dn-+l). It contains S?
as a strong deformation retract: simply collapse the finite telescope X(r) onto
this terminal sphere. Hence H,(X(r);Z) vanishes in degrees i ? ?,?, and so
#»(Sp;Z) vanishes in these degrees as well. The inclusion X(r) C X(r + 1)
sends [S?] to A;r+1[5^+1]. Thus an isomorphism Hn(S?;Z) = Jk is given by
mapping each [5?] to ( ? Ay·).
When ? > 2, S? is simply connected since its cells, aside from the base point,
all have dimension ? or ? + 1 (apply the Cellular approximation theorem 1.2).
Thus S?,n > 1, is indeed a "P-local space.
When ? = 1,5^ is an Eilenberg-MacLane space of type (A-, 1). Indeed any map
¦j -> S\> has image in some X(r), by compactness. Since X(r) ~ S1 it follows
that wmEj,) = 0, m > 2 and ???E^) is abelian. Thus tt^SJ,) = Hi(S$>) = Jk.
104 9 "?—local and rational spaces
Relative CW-p complexes are built by assembling "P-local disks via attaching
maps from "P-local spheres in exactly the way classical relative CW complexes
are constructed from ordinary disks. More precisely,
Definition A relative CW-p complex is a topological pair (X,A) together with
oo
a filtration A' = |J X(n) of X by closed subspaces ?(?) such that
n=-l
• X(i) = X(o) = X(-i) = A, and A is 1-connected.
• For ? > 1, X(n+i) is presented as
Xin+1) = lWU/n(Uo^), (9.1)
a
with /„ : LJ ? Sv,a -» x(n) a cellular map. The D^ are called the V-
local (n + l)-cells of (X, A). If A = {pi} then X is a CW-p complex. When
]k — Q we refer to (relative) CWq .
Remark Since S? is a subcomplex of the CW complex D^+1 and since /„ is
cellular, the adjunction space X(n) U./„ ( LI Q D^+l ) carries an induced structure
as a relative CW complex with respect to A. This makes {X, A) into a relative
CW complex in which each X(n) is a subcomplex. Since (Dv+l,Sj,) has only
(n + l)-cells and (n + 2)-cells, it follows that
Xn C X(n) C Xn+i , ? > -1,
where Xn is the ?-skeleton of the relative CW complex (A', A). We call X(n) the
?-skeleton of the CW-p-structure on (X, A). In particular (X,A) has no 0-cells
or 1-cells and so every circle in X is homotopic to a circle in the 1-connected
space A; i.e., X is simply connected.
Finally, as follows from the calculation above of i7»(S?;Z), ?,{??+? ,S?;Z)
is a free l:-module concentrated in degree n. It follows by excision that each
Ht(X(n+i),?(?);?) is "P-local. The long exact homology sequences now shows
that H,(X,pt;Z) is "P-local: thus X is a P-local space.
We turn now to the statement and proof of Theorem 9.3. This will make
frequent use (without reference) of the following elementary remark.
Lemma 9.2 Let ?p be the prime field of characteristic p. Then for all pairs of
spaces (X,A):
H.(X,A;Z) is V - local ?=> H.(X,A;Fp) = 0, p$V.
In particular
H.(X,pt;Z) is V- local ?=? H.(X;Fp) = H.(pt;Fp), ? $ V.
Homotopy Theory 105
proof: Consider the long exact homology sequence associated with the short
exact sequence
0 -> C.(X,A;Z) -^ C.(X,A;Z) —»· C,(X,A;?P) —»· 0.
It shows that multiplication by ? in H,(X,A;Z) is an isomorphism precisely
,(A',^;Fp) = 0. ?
Theorem 9.3 Let X be a simply connected topological space. Then the follow-
ing conditions are equivalent:
(i) ??,(?) is V-local,
(ii) H.(X,pt)Z) is ?-local.
(Hi) H,(QX,pt',Z) is V-local.
proof: This is essentially identical to the proof of Theorem 8.6. We begin, as
in that Theorem, with the case of Eilenberg-MacLane spaces, where we follow
closely the steps of the proof of Proposition 8.8:
Lemma 9.4 If X is an Eilenberg-MacLane space of type (?, ?), ? > 1, then
? is V-local =» H, (X; Fp ) = H, (pt; Fp ), ? ? V .
proof: Suppose first that ? = 1. Reduce to the case ? is finitely generated
exactly as in Case 3 in the proof of Theorem 8.6. Since Jk is a principal ideal
domain, finitely generated ^-modules are the finite direct sums of cyclic Jk-
modules. Hence in this case X has the weak homotopy type of a finite product
of spaces K(Ti, 1) with ?; a cyclic J;-module. Exactly as in the proof of Case 3
in (8.6) we are reduced to the case ? is a cyclic ^-module.
If ? = k then X has the weak homotopy type of Sj> and Ht(X,pt; Z) is V-
local by the calculation above of the homology of 5^>. If ?? ?. k then there is a
short exact sequence of ^-modules of the form
0—>¦&—>¦!;—»-?—»-?.
Exactly as in Case 1 of Proposition 8.8, this leads to a morphism of QF-fibrations
of the form
?.? ?{ ) UE
??
J.
pt
106 9 ?7—local and rational spaces
in which both UF and QE are Eilenberg-MacLane spaces of type (k, 1). Thus
as observed above both QF and ClE have the weak homotopy type of Sp. Hence
H,(QF;Fp) = Ht(QE;Fp) = Ht(pt,Fp). Thus Theorem 8.5 asserts that
Ht(pt;?p) A Hm{UK(n,2);Fp). Since ??'(??,2) has the weak homotopy type
of X, the Lemma holds for X.
This establishes Lemma 9.4 for Eilenberg-MacLane spaces of type (?, 1). The
case when X has type (??,?), ? > 1, follows by induction on n; apply Theorem
8.5 to the morphism of ??-fibrations
PX
pt > X.
We now return to Theorem 9.3, whose proof mimics the proof of formula (8.9),
with X replacing F and Fp replacing Jk. Thus we construct a sequence of spaces
{Xr}r>i starting with a cellular model ?? -> X, and a sequence of maps
er:Xr^Kr+u Kr+1 = K(nr+1(Xr),r + l).
The space Xr is r-connected, ??+?(??) is the identity isomorphism and Xr+i is
a cellular model for Xr *Kr+1 PKr+i. From Qr we also obtain the morphism
Xr XKr+1 PKr+i PKr+l
(9.5)
Xr — " Kr+\
of Q/<"r+i-fibrations and, as in the proof of (8.8), sequences of isomorphisms
nr+i(Xr) ^> TTr+l(Xr-l) ^> ^> 7Tr+l(^)·
Suppose H*(X,pt;Z) is TMocal. Then also if»(Zr,pi;Z) is TMocal for all
r, by induction. Indeed if this holds for some r then ??+1(??) is TMocal and,
by (9.4), H,(Kr+l;Fp) = H,(pt;Fp), p?V. Apply Theorem 8.5 to (9.5) to
conclude that H,(§r;Fp) is an isomorphism, ? ? V. Hence H*(Xr+l,pt;Fp) =
0, ? <? V, and H,{Xr+upt\ Z) is P-local. In particular each ???+1 (Xr) is "P-local
and so ?*(?) is 'P-local.
Conversely if ?» (?) is V-local the homotopy group isomorphisms above im-
nlv that each ???+1(?'?) is TMocal. Hence, by Lemma 9.4, H,(ClKr+i;Fp) =
Homotopy Theory
H*(pt;Fp) for r > 0 and ? g V. Regard
Xr *Kr+i PKr+l -
Xr
107
Xr
as a morphism from an {e}-fibration to an ???+? fibration. Conclude from
Theorem 8.5 that iJ»(pr;Fp) is an isomorphism for ? (? V. Since Xr is re-
connected, the sequence of isomorphisms
implies that H.(X;FP) = Hm(pt;Fp),p ? V\ i.e., H.(X,pt;Z) is TMocal.
This shows that (i) <?=> (ii). Suppose (iii) holds. Let j : {xo} -> X be the
inclusion of a basepoint and apply Theorem 8.5 to the map Pj : P{xo] —> PX
of path space fibrations to conclude that ii»(j;Fp) is an isomorphism for ? ? V.
Thus (iii) => (ii).
Conversely, suppose (i) and (ii) hold. Recall from §2(c) that there is a fibration
and a homotopy equivalence Y ~ X\ which identifies q with ?\. Since X\ ? ?2
??? is 2-connected and ^i{X\ ??2 ??) 5? ??(??), i > 3 these groups are, in
particular, 'P-local and we may apply (i) «-» (ii) to deduce that
?. (?(?? ??2 PK2);FP) = H*(pt;Fp) , ? i V .
Now note that iiq is an ?(?? ??2 -PA)-fibration. We shall apply Theorem 8.5
to
9
{pt}
{pt}
UY
Lemma 9.4 asserts that if»(/;Fp) is an isomorphism for ? ? V. Hence so is
H.faFp) and H,(UX;FP) S H.(ClXi;Fp) S H.(iiY;Fp) Si H.(pt;Fp), ? i V.U
(b) Localization.
Suppose ? : X —y Y is a continuous map between simply connected topologi-
cal spaces, and that Y is TMocal. Then ?»(?) is a Jfc-module and ?,(?) extends
uniquely to a morphism ?.(?)
108 9 "?—local and rational spaces
Definition A V-localization of a simply connected space X is a map ? : X —?
X-p to a simply connected P-local space X-p such that ? induces an isomorphism
When h = Q this is called a rationalization and is denoted by X —> X<q.
Theorem 9.6 A continuous map ? : X —> Y between simply connected spaces
is a V-localization if and only if Y is V-local and ?*(?;?) is an isomorphism.
proof: Since Y is P-local, 7r»(F) = ?*(?) ®z Ik and the homomorphism
?*(?) <8>z & —> ?.(?) is just ? »{?) <8>z Jt. Thus Theorem 9.6 is a special case of
the Whitehead-Serre Theorem 8.6. ?
Corollary The inclusion Sn —> SC, of the initial sphere in the telescope is a
V -localization. ?
Localizations always exist, and have important properties. We first describe
this with a cellular construction that gives the true geometric flavour of the
meaning of P-localization.
Example Cellular localization.
Suppose (X, A) is a relative CW complex in which Xy = Xo = A and
A is a simply connected P-local space. Thus the Cellular approximation the-
orem implies that X is simply connected. We shall use the natural inclusion
(Dn+1,Sn) —>· (?>p+1,S?) to construct a P-localization of X of the form
such that
• (X-p, A) is a relative CW-p complex.
• The P-local ?-cells of (X-p, A) are in 1 — 1 correspondence with the n-cells
of (X,A),n> 2.
• ? restricts to the identity in A, and to maps ?? : Xn —>· (X-p)^ satisfying
??+1 = ?? U ( IJ ??) : ?? U/n ( U D2+1) -> (??)(?) Us„ ( U D^),
? ? ? '
?? denoting the standard inclusion D^+l —> D^.
• In particular, ? is the inclusion of a sub relative CW complex.
In fact, suppose inductively that (Xv)(n) and ?? are constructed, and let
fa : S? —» A'n be the attaching map for some (n + l)-cell of (X,A). As noted
in Remark 9.1, (??>)(?) is P-local; thus ipnfa represents an element in the lk-
module ???((?-?){?)).
Homotopy Theory 109
We use this to extend ??/? to a cellular map ga : Spa —> (AV)(n)· Recall
CO OO
that S? = V S? Uh (UZi D]+1). Define ga : V ?>" —> (A»(n) so that pQ
i=0 i=0
restricts to </?n/a in S? and restricts to a representative of (?[=? ^j)^/«*]
in S", r > 1. (Here the kj are the integers prime to V used to define the
telescope). Since h attaches ?>"+1 by a map representing [S"_j] — kr[S"}. gah
is homotopically constant and so ga extends to all of S%>. Use the Cellular
approximation theorem 1.2 to replace ga by a cellular map, homotopic rel SJ to
the original.
Set gn = Uaga. Define (A»(n+1) = (A»(n) USn (IIQ ?>?"?) and define
??+? by the formula above. This completes the construction of ? : (A'. A) —>
(AV) A). To see that ? is a P-localization, it is enough to prove ?*(?\ k) is an
isomorphism (Theorem 9.6). This follows at once from the construction and the
fact that the standard inclusions induce isomorphisms
?? ( r\n + l en. il-i _5L ET ( n^+l Cn . n~\ ?
Theorem 9.7
(i) For each simply connected space X there is a relative CW complex (?-?,?)
with no zero-cells and no one-cells such that the inclusion ? : X —> X-p is
a V-localizatioin.
(ii) If (?-?,?) is as in (i) then any continuous map f from X to a simply
connected V-local space ? extends to a map g : X-p —> Z. If g' : X-p —> ?
extends f : X —> ? then any homotopy from f to f extends to a homotopy
from g to g'.
(iii) In particular, the V-localizations of (i) are unique up to homotopy equiv-
alence rel X.
proof: (i) Let ? : Y -> A' be a weak homotopy equivalence from a CW complex
Y such that Yi = Yo = {pt}. Let j : Y -» Y-p be a cellular ^-localization as
described in the Example above. Put
X-p = XU-? (? ? /) ?,· YVi
where we have identified (y,0) with 4>(y) and (y,l) with j(y). Then (X-p. X) is
a relative CW complex with no zero- or one-cells. Since A is simply connected
the Cellular approximation theorem implies that so is X-p.
Next use excision and the fact that ?*(?\?) is an isomorphism to deduce
H,(X-p, Y-p; Z) Si H.(X U^, Y x /, Y x {1}; Z) = 0.
Thus i/.(A>,pi;Z) Si H.(Yp,pt;Z). Since Y-p is TMocal so is Xv.
HO 9 ?7—local and rational spaces
Finally, since j is a "P-localization we may use excision to deduce that
H.{Xv,X;k) = Hm(Yp,Y;Jk) = 0.
Hence the inclusion ? : X -> X-p satisfies ??{?;??) is an isomorphism; i.e., ? is
a ^-localization.
(ii) Let (ZU/ X-p, Z) be the relative CW complex obtained by identify-
ing ? ~ f(x),x € X- Since ? is V-local the construction of the Example above
gives a ^-localization
a:(ZL)fX-p,Z) -> ((Z Uf XV)V,Z).
In particular, ?*(?,?) is an isomorphism.
On the other hand, ?*(?\ Jk) is an isomorphism, and hence H*(ZL)f X-p, Z; Jk)
S H*{Xv,X;k) = 0. Thus H*(Z,Jk) A H,(Z Uf ??;?) and the composite
inclusion i : ? —)¦ (? U/ Xv)v also satisfies: Hm(i;]k) is an isomorphism. By
the Whitehead-Serre theorem 8.6, 7r»(i) ® J: is an isomorphism. Since ? and
(Z U/ Xp)p are 'P-local they satisfy ??»(—) = 7r»(—) (g! $:. Hence i is a weak
homotopy equivalence.
Now the Whitehead lifting lemma 1.5 can be applied to
? U/ Xv ) (Z U/ .Yp)p
to obtain a retraction r : Z U/ Xp -> ?. Define ? to be the composite X-p ->
ZU/IpA Z.
Finally, suppose p' : Xp -> ? restricts to /' ·. X -> ? and ?: XxI-^Zsa
homotopy from / to /'. Consider the map
V1 = @,?,0')= (Xpx{0})UIx/U(IPx{l})->Z.
An easy calculation like those above shows that the inclusion (X-p ? {0}) U ? ?
lu (A> x {1}) -» ??? ? J is a 7^-localization. Hence ? extends to a homotopy
from g to g'. ?
(c) Rational homotopy type.
We specialize now to the case Jk = Q and V = ?. Consider a continuous map
/: X->Y
between simply connected spaces. It follows from the Whitehead-Serre theorem
8.6 that the following conditions are equivalent:
• vr»(/) ® Q is an isomorphism.
• .#*(/; <Q>) is an isomorphism.
Homotopy Theory 111
• H*(f;Q) is an isomorphism.
In this case / is called a rational homotopy equivalence .
Theorem 9.8 implies that the rationalizations Xq of a simply connected space
all have the same weak homotopy type and that the weak homotopy type of Xq
depends only on the weak homotopy type of X.
Definition The weak homotopy type of Xq is the rational homotopy type of
X.
Theorem 9.7 also gives a second, equivalent description of rational homotopy
type that does not explicitly involve rationalization.
Proposition 9.8 Simply connected spaces X and Y have the same rational
homotopy type if and only if there is a chain of rational homotopy equivalences
X <— Z@) —>···«— Z(k) —> Y.
In particular if X and Y are CW complexes then they have the same rational
homotopy type if and only if Xq ~ Yq.
proof: The first assertion is immediate from Theorem 9.7. The second follows
immediately from the Whitehead lifting lemma 1.5, because (Xq, X) and (Yq, Y)
are relative CW complexes. ?
Note: Rational homotopy theory is the study of properties of spaces and maps
that depend only on rational homotopy type; i.e.. are invariant under rational
homotopy equivalence.
A rational cellular model for a simply connected space Y is a rational homotopy
equivalence ? : X -> Y from a CW complex X such that ?? = Xo = {pt}- We
complete this section by observing, for simply connected spaces Y, that
• H*(Y;Q) has finite type <=> Y is rationally modelled (9.9)
by a CW complex of finite
type
• H,(Y;Q) is finite dimensional <?> Y is rationally modelled (9.10)
and concentrated in by a finite TV-dimensional
degrees < ? CW complex
Both these observations are corollaries of
Theorem 9.11 Every simply connected space Y is rationally modelled by a
CW complex X for which the differential in the integral cellular chain complex
is identically zero.
112 9 "?—local and rational spaces
Corollary Ht(X;Z) is a free graded Z-module.
proof of 9.11: With the aid of cellular models (Theorem 1.4) we reduce to
the case y is a CW complex, Yo = Y\ = {pi}, and all cells are attached by based
maps (Sn,*) -> (Yn,pt). Let (C»,<9) denote the cellular chain complex for Y
and use the same symbol to denote an ?-cell of Y and the corresponding basis
element of Cn ¦
Choose ?-cells a" and 6" so that in the rational chain complex (C» <g! Q. d),
Cn®Q = (kerd)n@0Qa? = (Im d)n ® 0 Qa? ? ? <Qb].
i i j
Define subcomplexes W(n) C Z(n) C Yn by
W(n) = Yn^U(\Jaf) and Z(n) = W(n)u(\Jb]).
i j
Since d : ©Qof —*¦ (Im d)n-i the Cellular chain models theorem 4.18 asserts
that
H,(W(n),Z(n - 1);Q) = 0. Thus the inclusion ? : ? (? - 1) -> W(n) gives an
isomorphism of rational homology, and the Whitehead-Serre theorem 8.6 shows
that ?, (?) <g> Q is an isomorphism. In particular, since the cells b^ are attached
by maps /_,· : (S",*) -» Yn-\ C W(n), there are maps g^ : (Sn~1,*) -»
(Z(n — 1), *) and non-zero integers rj so that
7??-?(?)[&] = rjlfj].
We now construct ? : X -> Y inductively so that ? restricts to rational
homotopy equivalences ?? : Xn -> Z(n). Begin with X1 = Xo = {pt} and
?? : ?? -» Yi = {pi}. Suppose ??-? ¦ Xn-\ ->· ^(" — 1) is constructed. Then
there are maps hj : (S",*) -> (Xn_1;pi) and non-zero integers Sj such that
7??_?(???_?)[^] = Sj[gj}. Set ? = {hj} : \fj S]1 -> Xn_i, and set
Choose maps oj : (Sn~1,*) -> (S", *) such that [??] = s^r^S71'1] in
*n-i(Sn-\*). Thus in ?^???^?),*) we have
[t follows that fjOj is based homotopic to </?n_i/ij; i.e. there are maps
?, : S-1 x [|, 1] -> W(„), with j *["· ^ = ^
Homotopy Theory
Then write
Z(n) = W{n)Uf{\jD?), f = {/;} : \/S?-1 -> Yn-U
5
and extend ??-? to ?? by setting
$j(v)t)eW(n), veS?-\ ?<?<1,
Put X'n = Xn-i Uh (V-5" x [5,1])· Then {Xn,X'n) is a relative CW
complex whose cells are the disks DJ(^) of radius ^. An immediate cellu-
lar chains argument (Theorem 4.18 and the following Remark) shows that ??
induces a homology isomorphism H,(Xn,X'n;Q) A H+(Z(n), W(n);Q). But
Xn-i is a deformation retract of X'n-i, an<^ tne maps ? : Z(n — 1) -> It7(n) and
(/?n-i ^ -Xn-i —> Z(n— 1) induce isomorphisms of rational homology- Hence
which gives
?.{??) ¦¦ H.{Xn,ty A ii.(Z(n);Q)
as desired.
Finally, observe that for any n. Hn(Z(n): Q) -=> Hn{Z{n),Z{n-1); Q). by an
immediate cellular chains argument. Hence iin(Xn;Q) ^» iin(Xn,Xn_i;Q). If
(Cx, O) denotes the integral cellular chain complex for X then this implies that
(C<nld) ® Q -> (^;0) (S) Q yields an isomorphism of homology in degree n.
This implies <9 : C% -> C^Lj is zero, again, for all ?. ?
Exercises (All spaces are supposed 1-connected.)
1. Determine X(p) for ? = 2,3 or 0 and X = Sn ?? Dn+1 with ? : S" -> S"
a continuous map of degree 6 (resp. 5). Same question with the fibre of the
collapsing map X -> S"+1.
2. A finite complex X is universal if for any rational equivalence ? : A —> ?
and continuous map f : X -> ? there exists a rational equivalence ? : X -> X
and a continuous map g : X -> A such that ? ? g ~ / ? ?. Let X and F be
finite complexes and / € [Xq, Yq\. Prove that if X is universal then there exists
g 6 [X, Y] and a rational equivalence a : X -> X such that gq ~ / ? aQ.
3. Let Pbea set of prime numbers, V C V, and define the ring k' by ifc' =
{??/?: | fc is relatively prime to the elements of V'}- Consider two localization
maps / : X -> Xv and /' : Y -> Yv> with 7>' c V- Prove that if / : X -> F
!S a continuous map then there exists a map j\^> : X-p —> Yp», unique up to
homotopy. such that //_/< ? / = /' ? / and the following diagrams commute:
114 9 ?7—local and rational spaces
Kn(X)®Ik' n"-^id Kn{Y)®Ik' Hn{X)®Ik' H"Md Hn{Y)®Ik'
s*| |~ =1 l^
nn(Xv)®Ik> ?^>" ??(??.) Hn(Xv;Ik') "^ Hn(Yv.)
4. Let V be a set of prime numbers and the ring Ik be as defined at the beginning
of this section. A continuous map / : X -> Y is a V-equivalence if for any ? > 2,
iTn(X)®Bi *"-—> irn(Y)®Ik is an isomorphism. Prove that / is a ^-equivalence
if and only if f-p is a homotopy equivalence. When Y is supposed 2-connected,
prove that this is also equivalent to the following assertion : ?/ : CIX —> QY is a
^-equivalence. Remark: It is not true that the existence of a p-equivalence f :
X —> Y for every prime ? implies that ? ~ ?. This motivates the introduction
of the genus of a space X, i.e. the set of [Y] such that for any prime p, Yp ~ Xp.
5. Let / : X -> Y be a rational homotopy equivalence between finite CW
complexes and V be the set of all primes. Prove that there exist p\ ,??,...,?^ € V
such that Xp ~ Yp where ? = V — {p\,pi, ¦¦¦•.Pk)-
Part II
Sullivan Models
10 Commutative cochain algebras for spaces and
simplicial sets
In this section the ground ring is a field k of characteristic zero.
Recall from §5 that C*(X; k) denotes the cochain algebra of normalized singu-
lar cochains on a topological space X. This algebra is almost never commutative,
although it is homotopy commutative.
Now however, because k is a field of characteristic zero, it turns out that we
may replace C*(X; k) by a genuinely commutative cochain algebra. More pre-
cisely, we introduce a naturally defined commutative cochain algebra Apl (X; k),
and natural cochain algebra quasi-isomorphisms
C*(X;k) ^ D(X) <?- APL(X;k),
where D(X) is a third natural cochain algebra. The construction of
ApL(X',k) , due to Sullivan [144], is inspired from C°° differential forms, while
reflecting the combinatorial nature of how the singular simplices of X fit together.
The functor
X^ APL(X;k)
serves as the fundamental bridge which we use to transfer problems from topology
to algebra. It is important to note that this functor is contravariant; i.e., it
reverses arrows.
The quasi-isomorphisms above will be constructed in Theorem 10-9. They
define a natural isomorphism of graded algebras,
H*(X;k) = H(APL(X;k)), A0.1)
and we shall always identify these two algebras via this particular isomorphism.
Thus for any continuous map, f, we identify H*(f;k) = H(ApL(f;k)).
Recall now from Proposition 9.8 that two simply connected spaces X and Y
have the same rational homotopy type if and only if there is a chain of rational
homotopy equivalences
X <— Z@) —>···¦«— Z(k) —> Y.
There is an analogous notion for commutative cochain algebras: two commuta-
tive cochain algebras (.4, d) and (B, d) are weakly equivalent if they are connected
by a chain
(A,d) ^ (C@),d) ^ ··. -?> (C(k),d) ?- (B,d)
of quasi-isomorphisms of commutative cochain algebras. Such a chain is called a
weak equivalence between (A,d) and (B,d).
Next recall from §9(c) that a continuous map f : X -> Y between simply
connected spaces is a rational equivalence if and only if H*(f;Q) is an isomor-
phism. Since k is a field of characteristic zero and we identify H(ApL(f;k))
116 10 Commutative cochain algebras for spaces and simplicial sets
with H*(f;]k) it follows that f is a rational equivalence if and only if ApLJ(f;Jk)
is a quasi-isomorphism.
In particular if X and Y have the same rational homotopy type then Api (X; Jk)
and Api(Y;lk) are weakly equivalent, and so Apl(—;Jk) defines a map
rational homotopy
types
? f weak equivalence classes of ?
j [ commutative cochain algebras. J
In §17 we show that if h = Q and if we restrict to simply connected spaces X
and commutative cochain algebras (A,d) such that H*(X:Q) and H(A,d) have
finite type, then this correspondence is a bijection. This fundamental result of
Quillen-Sullivan reduces rational homotopy theory to the study of commutative
cochain algebras. As noted in the Introduction, our purpose in this text is to
show how this result may be applied to extract topological information from the
algebra.
Since homotopy information is carried by the weak equivalence class of
ApL(X;Jk) we may (and frequently will) replace it by a simpler, weakly equiv-
alent, commutative cochain algebra: such a cochain algebra will be called a
commutative model for X. More formally we make the
Definition: A commutative cochain algebra model for a space X (or simply a
commutative model for X) is a commutative cochain algebra (A,d) together with
a weak equivalence
Henceforth we shall fix is and, for the sake of simplicity suppress Is from the
notation, writing Api(-) for Api(-;Js) and C*(-) for C*{-;h). The rest of
this section is spent on constructing APi{X) and establishing its relation to
C*{X). The section covers six topics:
(a) Simplicial sets and simplicial cochain algebras.
(b) The construction A(K).
(c) The simplicial commutative cochain algebra ApL, and
(d) The simplicial cochain algebra Cpl, and the main theorem.
(e) Integration and the de Rham theorem.
(a) Simplicial sets and simplicial cochain algebras.
A simplicial object ? with values in a category C is a sequence {Kn}n>o
objects in C, together with C-morphisms
* ?. D< i < ? + 1 and ss : Kn -> Kn+1, 0 < j < n.
Sullivan Models 117
satisfying the identities
, ?
, i
, i
, i
<;;
<i;
= Ji
>;
+
+ 1
1.
id
A simplicial morphism f : L —> ? between two such simplicial objects is a
sequence of C-morphisms ?? : Ln -> Kn commuting with the 9* and Sj.
A simplicial set ? is a simplicial object in the category of sets. Thus it
consists of a sequence of sets {Kn}n>o together with set maps di,Sj satisfying
the relations A0.2). The motivating example is; of course, the simplicial set
S*(X) = {Sn(X)}n>o of singular simplices ? : ?" —> X on a topological space
A', with face and degeneracy maps are defined in §4(a). This is a functor, with
a continuous map / inducing the morphism S*(f) : ? \—> f ? ?, ? € Sn(X).
A simplicial cochain algebra A is a simplicial object in the category of cochain
algebras: it consists of a sequence of cochain algebras {An}n>o with appro-
priate face and degeneracy morphisms. Similarly simplicial cochain complexes,
simplicial vector spaces ... are simplicial objects in the category of cochain com-
.plexes, vector spaces, Thus a morphism of simplicial cochain complexes,
? : D -* E, is a sequence ?? : Dn -> En of morphisms of cochain complexes,
compatible with the face and degeneracy maps. If each ?? is a quasi-isomorphism
we call ? a quasi-isomorphism of simplicial cochain algebras.
Let ? be any simplicial set. By analogy with St(X) the elements ? € Kn
are called n-simplices and ? is non-degenerate if it is not of the form ? = sjt
for some j and some r € Kn^\. The subset of non-degenerate n-simplices is
denoted by NKn. It follows from the relations A0.2) that for each ?· > 0 a
subsimplicial set K(k) C-K is given as follows: for ? < k, K(k)n = Kn and for
? > k, K(k)n = {sjt | 0 < j < ? - 1, r€ /f (fc)n-i}· The simplicial set K(k) is
called the k-skeleton of K, and is characterized by
??? , n<k
? \nlk.
The dimension of ? is the greatest k (or oo) such that NKk ? ?, and this is
also the least k such that K(k) = K.
Finally, we use the notation of §4(a) to introduce a sequence of important sub-
simplicial sets ?[?] C St (?"), ? > 0. Let e0,... , en be the vertices of ?". Then
^¦[n]k c Sk(An) consists of the linear fc-simplices of the form ? = {eio · · ¦ eik)
with 0 < i0 < · · · < ik < n. Thus ? is non-degenerate if and only if i0 < · · - < i*.
In particular A[k] is fc-dimensional and the identity map
cn = {e0 ¦ ¦ ¦ e„> : ?" -> ?"
•s the unique non-degenerate ?-simplex; cn is called the fundamental simplex of
118 10 Commutative cochain algebras for spaces and simplicial sets
?[?]. The (n— 1)-skeleton of ?[?] is denoted by dA[n] and is called the boundary
of ?[?].
Lemma 10.3 //if is any simplicial set then any ? € ??? determines a unique
simplicial set map ?» : ? [?] -> ? such that a»(cn) = ?.
proof: The verification using A0.2) is straightforward, but the reader may also
refer to [122]. ?
A simplicial object A in a category C is called extendable if for any ? > 1 and
any 1 C {0,...,n}, the following condition holds: given ?* € An_i,i € 2, and
satisfying
9???· = 97·_??? ,i<j,
there exists an element ? e An such that ?* = oj-?, i € 2.
(b) The construction A(K).
Let J\~ be a simplicial set, and let A = {An}n>0 be a simplicial cochain complex
or a simplicial cochain algebra. Then
A(K) = {Ap(K)}p>0
is the "ordinary" cochain complex (or cochain algebra) defined as follows:
• AP(K) is the set of simplicial set morphisms from ? to Ap.
Thus an element ? G AP(K) is a mapping that assigns to each n-simplex
? e Kn (n > 0) an element ?? € (Ap)n, such that ??;? = ???? and
• Addition, scalar multiplication and the differential are given by
(? + ?)? = ?? + ??, (?·?)? = ?·?? and
• If A is a simplicial cochain algebra multiplication in A(K) is given by
(?·?)? = ??·??.
• If ? : ? -> L is a morphism of simplicial sets then A(ip) : A(K) < A(L)
is the morphism of cochain complexes (or cochain algebras) defined by
(?(?)?)? = ???.
• If ? : A -> ? is a morphism of simplicial cochain complexes (or simplicial
cochain algebras then ?{?) : A(K) -> B(K) is the morphism defined by
(?(?)?)? = ?{??).
Sullivan Models 119
• When X is a topological space we write A(X) for A(S*(X)).
• If ? € Kn then ? ?-»· ??.defines a morphism A(K) —> An called restriction
to ?.
Remark Note that the construction A(K) is functorial in A and contrafunc-
torial in K.
Proposition 10.4 Let A be a simplicial cochain algebra.
(i) For n> 0 an isomorphism A(A[n\) —=1—» An of cochain algebras is given
by ? ?—> $c„> where cn is the fundamental simplex of ?[?].
(ii) If A is extendable and L C ? is an inclusion of simplicial sets, then
A(K) -> A(L) is surjective.
proof: (i) The definitions show that ? ?—> ??? is a morphism of cochain
algebras. Since ?.?(?[?]) consists of the simplicial set maps ?[?] -> Ap, Lemma
10.3 asserts that this morphism is a bijection.
(ii) Given ? € A(L) we give an inductive procedure for constructing
? € A(K) that restricts to ?. In fact, suppose we have found elements ??, ? €
Kk,
k < ? such that
?? = ??, ? ? Lk; ?9:? = ?{??; <eSjT = Sj-?,-, r e Km, m < ? - 1.
Then define ??,? € ?? as follows.
If ? € Ln, ?? = ??. If ? has the form Sjt, ? e Kn-i, use A0.2) to see that
5_;?? is independent of the choice of j and r and put ?? = Sj$r. Finally, if
? € Kn — Ln is non-degenerate note that di($QjC) = dj-i($dia)A < j- Since A
is extendable we may therefore choose ?? so that ?*?? = ??,.?, 0 < i < ?. In all
cases the equations above will be satisfied, as follows easily from A0.2). D
If A is an extendable simplicial cochain algebra and L C ? is an inclusion
of simplicial sets we denote by A(K, L) the kernel of the surjective morphism
A{K) -> A(L).
Thus A(K, L) is a differential ideal in A(K) and
0 -> A(K,L) -> A{K) -> A(L) -> 0
is a short exact sequence of cochain complexes, natural with respect to (K, L)
and with respect to the simplicial cochain algebra A.
A key step in the proof of Theorem 10.9 is the following
Proposition 10.5 Suppose ? : D -> ? is a morphism of simplicial cochain
complexes. Assume that
120 10 Commutative cochain algebras for spaces and simplicial sets
(i) H{?n) : H(Dn) -> H (En) is an isomorphism, ? > 0.
(ii) D and ? are extendable.
Then for all simplicial sets K,
?(?(?)) : H(D(K)) -> H(E(K))
is an isomorphism.
First we establish a preliminary lemma. Define
a:A(K(n),K(n~l)) > JJ A(A[n],A[n - 1]) ,n>0,
by setting a : ? ?-)· {^4(?,)?}?6^//<·?, where ?» : ? [?] -+ ? is the unique
simplicial map (Lemma 10.3) such that a»(cn) = ?.
Lemma 10.6 If ? is a simplicial set and A is an extendable simplicial cochain
complex then a is an isomorphism, natural in A and in K.
proof: If ?(?) = 0 then ? vanishes on all the non-degenerate simplices of ? (?)
and hence ? = 0. Conversely, given a family {?? € ?(?[?],?[? — 1])}?&???,
we recall first that (Proposition 10.4 (i))
A(A[n],A[n-l])cA(A[n}) = An.
This identifies the ?? as elements of An satisfying 9??? = 0, 0 < i < n. Define
? € A(K(n),K(n — 1)) by the three conditions:
{?? =0 , ? G Km, m < ?
?? =?? , ?????
? s ja = Sj-?? , all i, ?.
Clearly ?? = {??}. D
proof of 10.5: For any inclusion L C M of simplicial sets we have the row
exact (Proposition 10.4 (ii)) commutative diagram
0 D(M,L) — D(M) D(L) >- 0
9(M,L)
?{?)
e(L)
E(M, L) — E(M) E(L)
Sullivan Models 121
Hence, if any two of the vertical arrows is a quasi-isomorphism, so is the third.
Moreover Proposition 10.4 (i), identifies 0(?[?]) : ?>(?[?]) -> E(A[n\) with ?? :
Dn -> ?1,,. Hence ?(?[?\) is a quasi-isomorphism, ? > 0. We now use induction
on ? to show that for any simplicial set ?, ?(?(?)) is a quasi-isomorphism.
Indeed, this is vacuous for ? — —1. Suppose it holds for some ? - 1. From
Lemma 10.6 and the remarks above it follows that ?{—) is a quasi-isomorphism
when (—) is in turn given by:
dA[n], (?[?], dA[n}), (K(n), K(n - 1)) and K{n).
This completes the inductive step. Along the way we have also shown that each
?(?(?),?(? — 1)) is a quasi-isomorphism.
Finally, let ? be any simplicial set. Given ? e D(K) and ? € E(K) such
that ?? = 0 and 0(??)? = d*, we shall find ? e D(K) and ? € E(K) such
that ? = du and ? = ?(?)? + dF. This implies at once that ?(?) is a quasi-
isomorphism (Lemma 3.2).
To find ? and ? we construct inductively a sequence ?? e D (K, K(n — 1))
and ?? e ? (?, ? (? - 1)) such that
and
? - ? (e(K)iii + dTi) ? ? (?, ? (ri)).
Indeed, set ?_? = ?_? = 0. If ?; and I\- are constructed for i < n, set
?' = ? - ? dn{ and ?' = ? - ? (?(?)??? + dTi). Then ?' restricts to
?" € D(K(n),K(n-l)), ? restricts^ ?" € ?(?(?),?(?-1)), d$" = 0
and ?(?)?" = d*". Our remarks above show that ?(?(?),?(? - 1)) is a
quasi-isomorphism. Hence we can find ?" € D (K(n),K(n — 1)) and ?" €
E(K(n),K(n - 1)) such that ?" = du" and ?" - ?{?)?." + ??". Now close the
induction by using the extendability of D and ? to find ?? € D (K, K(n — 1))
and ?? 6 E(K,K(n - 1)) which restrict respectively to ?" and ?".
Finally, the sequence {??} satisfies (??)? = 0, ? > dim ?. Thus we may
define ? e D(K) and ? € E(K) by ?? = ?(??)? and ?? = ?(??)?. Clearly
? ?
? = dU and ? = 0(??)? + dr.
D
(c) The simplicial commutative cochain algebra Apl, and Apl(X).
The first step in constructing a functor from topological spaces to commuta-
tive cochain algebras is the construction of the simplicial commutative cochain
algebra, APL.
For this consider the free graded commutative algebra ?(?0, · · · , tn, y0,... , yn)
~"cf· §3, Example 6.- in which the basis elements U have degree zero and the
122 10 Commutative cochain algebras for spaces and simplicial sets
basis elements yj have degree 1. Thus this algebra is the tensor product of the
polynomial algebra in the variables U with the exterior algebra in the variables
yj. A unique derivation in this algebra is specified by U t-> yt and yj t-> 0. It
preserves the ideal /„ generated by the two elements ]T? U - 1 and J2o Vj-
Now define APL = {(APL)n}n>0 by:
• The cochain algebra (APL)n is given by
. _ A(t0,... ,tn,y0,... ,yn)
{APL)n - (sti_ijEyi) '
dti = yi and dyj = 0.
• The face and degeneracy morphisms are the unique cochain algebra mor-
phisms
di : (APL)n+i -> (APL)n and Sj : {APL)n -> (APL)n+i
satisfying
{tk ,k<i ( tk ,k <j
0 ,k = i and sj : tk ?—> < tk + tk+i ,k = j
[
Notice that the inclusions U -> (APL)n,yj -> (APl)ti extend to an isomorphism
of cochain algebras,
(?(*?,... ,tn,yu... ,yn),d) A (APL)n.
Remark 1 The elements of (APl)ti are called polynomial differential forms
with coefficients in h, for the following reason. When Ac! (e.g. Jk = Q) the
algebra
(APL)°n = ?[??,...,??] /(Eif-1)
is the Jk- (eg. rational) subalgebra of the smooth functions C°°(A") generated
by the restrictions U of the coordinate functions of R"+1. This identifies {APl)ti
as a sub-cochain algebra of the classical cochain algebra, ????(??) of real C°°
differential forms on ?"; moreover, Adr(^") = C°°(An) ®(?? )? (APL)n.
Note that this also identifies
di = ADR(Xi) and Sj = Adr(qj),
where the Aj are the face inclusions and the ?3- are the degeneracies for the
simplices ?" as defined in §4(a). These ideas will be further developed in §11.
Remark 2 Each APPL, p > 0, is itself a simplicial vector space and APl =
{-4pL} >0, equipped with the obvious simplicial multiplication and differential.
Sullivan Models 123
Lemma 10.7
(i) (APL)o = k-\.
(n) H ((APL)n) = ?c ¦ 1, n>0.
(Hi) Each APPL is extendable.
proof: (i) This is immediate from the definition.
(ii) The isomorphism above identifies (ApL)n as the tensor product
® A(if)yt). Since d(i*) =kt*-1yi if follows that H(A(ti,yi)) = *· 1. But #(-)
commutes with tensor products (Proposition 3.3) and so H((Apl)ti) = ic · 1 as
well.
(in) Suppose given X C {0,... ,n} and elements ?* € (^???,)?-?,
? € I, satisfying ^?^- = dj-\$i,i < j. Beginning with ?_? = 0, we induc-
tively construct elements
*r € (Apl)ti, —1 < ? < n, such that:
oj*r = ?{, Hi el and ? < r.
We may suppose ??_? constructed. If r ^ 1 set ?? = ??_?; otherwise proceed
as follows. Embed the polynomial algebra {A0PL)n in its field of fractions F
and let B° C F be the subalgebra generated by (A°PL)n and the element ?^-.
Setting d ( y^- ) = ,^^? defines a cochain algebra
? = B°®{AopL)n(ApL)n=B°®A(dtu...,dtn)
which contains (/lpz,)n· Moreover a morphism ? : {ApL)n-\ -> ? ?? cochain
algebras is given by
and ?(???) = ap{ti).
On the other hand, we may extend dr to a morphism ? -> (.4??,)?_? by setting
dr (ji-) = 1. Clearly dr ? ? = id.
Every element of ? has the form -: -^? for some ? > 0 and some
A ir)
* e {ApL,)n- In particular we may write
A - ??)??(?? - ar*r_0 = ?, some ? € (APi)n.
Now, for i 6 X and i < r, we have
0;(?? - ar*r_0 = 3?_?(?{ - af*r_!) = 0.
124 10 Commutative co chain algebras for spaces and simplicial sets
Hence ?? — <9???_? is in the ideal generated by U and dti in (/lpz,)n-i, and it
follows that ? is in the ideal generated by U and dU in (ApL)n. In particular,
<9;? = 0, i e 1 and i < r.
On the other hand, since ??? = id, we also have 9?? = ?? - <9???_?. Thus
9;(? + ??-?) = $j for ; € X and j < r. This closes the induction. D
Because the graded algebras (???,)? are commutative, the construction Apl( )
defined in (b) assigns to each simplicial set ? a commutative cochain algebra,
Apl(K), and to each map / of simplicial sets a morphism, APl(/) of commu-
tative cochain algebras. Since Apl is extendable, with every pair L C ? of
simplicial sets is associated the short exact sequence
0 —> APL(K,L) —> APL(K) —> >ipL(L) —> 0.
For topological spaces X and continuous maps / we apply this construction
to the simplicial set S»(X) and to ?¦(/). This defines a contravariant functor
from spaces to commutative cochain algebras, which will be denoted
X~+APL{X) and / - APL(f).
In particular, associated with a subspace Y is the short exact sequence
0 -> APL(X, Y) -> .4PL(.Y) -> APL(K) -> 0.
When it is necessary to indicate the coefficient field explicitly we shall write
APL(X]Js), APL(K;Je) and APL(f;k) for APL(X),APL(K) and APL(f).
An element of APL{X) is a function assigning to each singular ?-simplex of
X a polynomial p-form on ?", ? > 0, compatible with the face and degeneracy
maps. This motivates the following terminology: The commutative cochain
algebra, APl(X), is the cochain algebra of polynomial differential forms on X
with coefficients in k.
Example 1 When X = {pi}, then S*(X) = ?[0]. It follows that APL{X) =
(APLH = Je-t
APL(pt) = M.
Thus an inclusion j : pt —> Y induces an augmentation ? = APl (j) : APl (Y) —>
A.
(d) The simplicial cochain algebra Cpl, and the main theorem-
Recall from §5 that with every topological space X is associated to its singular
cochain algebra, C*(X; 3c). Recall that we simplify notation and write C* (X) for
C*(X; &). Now the construction oiC'(X) depends only on the singular simplices
of X and their face and degeneracy maps; i.e., it depends only on the simplicial
set St(X). As such it generalizes to any simplicial set ? to give the cochain
algebra C*{K), also written C*(K; k) if we need to emphasize coefficients. More
Sullivan Models 125
• C*(K) = {Cp(K)}p>0\
• CP(K) consists of the set maps Kp —> fc vanishing on degenerate simplices.
• For / ? CP(K), g ? Cq(K) the product is given by
(/ U g){a) = (-l)p"f(dp+1 ¦ ¦ ¦ dp+qa) · g(dodo ¦ ¦ ¦ doa), ? G Kp+q.
• The differential, <2, is given by
P+l
(df)(a) = ?(-1)?+?+1/(?4?) ,? G Kp+I, f G CP{K).
i=0
Observe that, by definition, C*(X) — C*(S*(X)) for topological spaces X.
Next observe that a simplicial cochain algebra Cpl can be defined using the
simplicial sets ?[?] C 5.(?") introduced in (a). Indeed since the face inclusions
and degeneracy maps for the ?" have the form
Xi = (eo,---ei---en+i):An^An+1
and
Pi = <e0,··· ,eheh-·· ,en) : An+1 -> ??,
(cf. §4(a)) it follows that 5.(X{) and S*(pj) restrict to simplicial maps
[Xi] : ?[?] -> ?[? + 1] and [Pj] : ?[? + 1] -> ?[?].
Thus we define Cpl — {(CpL)n}n>o by
• (Cpl)? is the cochain algebra C*(A[n]).
• The face and degeneracy morphisms are the C*([Xi]) and C*([pj]).
Finally, let
® ApL = {(CpL)n ® (ApL)n\ 9j <8> <%,· Sj ® Sj }
be the tensor product simplicial cochain algebra (where (Cpl)u ® (Apl)u is the
tensor product cochain algebra described in §3(c)). Morphisms
Cpl —> Cpl ® Apl i— Apl
are defined by 7 t-> 7 0 1 and ?? 1 0 ?. Thus, for any simplicial set ? they
determine the natural cochain algebra morphisms
CPL(K) -> (CPL ®APL)(K) ^~ ApL(K).
Our main result, which now follows, is (together with its proof) due to Chris
Watkiss [155] following the idea in Weil's proof [156] that de Rham cohomology
and singular cohomology are isomorphic.
126 10 Commutative cochain algebras for spaces and simplicial sets
Theorem 10.9 [155] Let ? be a simplicial set. Then
(i) There is a natural isomorphism Cpi(K) -^ C*(K) of cochain algebras.
(ii) The natural morphisms of cochain algebras,
Cpl(K) -> {CPL®APL){K) <- APL(K)
are quasi-isomorphisms.
Substituting S*(X) = ? in Theorem 10.9 we obtain
Corollary 10.10 For topological spaces X there are natural quasi-isomorphisms
of cochain algebras.
C*(X) —?-> (CPL®ApL){X)^^—ApL{X) D
This gives the isomorphisms H*(X) = H(APL(X)) promised in A0.1).
For the proof of Theorem 10.9 we require two lemmas.
Lemma 10.11 There are natural isomorphisms Cpl{K) —> C*(K).
proof: Each 7 G CPL(K), ? > 0 determines the element / G CP(K) given by
/(?) = 7<r(cp) , ? G Kp,
where cp is the fundamental simplex of ?[?]. It follows, by a straightforward
calculation from the definitions and A0.2), that the correspondence 7 1—> f
is a cochain algebra morphism. To show it is injective, assume 7 1—> 0. The
elements a ? ?[?]? are the linear simplices of the form {eio,... ,e,· ) : ?? -> ?"
with i0 < ... < ip, and these can all be written as composites of face and
degeneracy maps. It follows that for any ? > 0 and any r G Kn,
Gr)(a) = Gr)(or O Cp) = Jroa(Cp) = 0.
Hence 7 = 0 and our morphism is injective.
On the other hand, by Lemma 10.3 any ? e Kn determines a unique simplicial
map ?. : ? [?] -> ? such that ?.(??) = ?. Thus, if / G CP(K), we may define
7 € CPL(K) by ?? = ??(?»)(/). Clearly 7 ?-> / and our morphism is surjective.
D
Lemma 10.12
(i) H(CPL)n = A = H((CPL)n 0 (APL)n), ? > 0.
(ii) CpL is extendable.
^ ?. .?^,? ;R extendable.
Sullivan Models 127
-proof: (i) The first assertion is the classical calculation of ?* (?[?]) which is
left to the reader; the second then follows from Lemma 10.7 (ii) and the fact
that H( —) commutes with tensor products (§3(e)).
(ii) The map [?*] : ? ?-> ?* ? ? is an isomorphism of the simplicial set
?[? — 1] onto a subsimplicial set F(i) C ?[?]. Thus it identifies a p-cochain
fi G Cp(A[n - 1]) with a set map // : F(i)p -> Je. Given a sequence /f,i G
X C {0, ...,n} of such cochains, the condition difj — dj-ifi, i < j, implies
that f[ and /j restrict to the same function in F(i)p ? F(j)p. Thus {/?} defines
a function from (J F(i)p to Ik, which then trivially extends to a function / in
?[?]?, vanishing on degenerate simplices. Thus / is an element in Cp(A[n]) such
that dif = fi,i el
(iii) Suppose given elements ?* G <??(?[? - 1]) 0 (^L)n_1} ielc
{0,... ,n}, satisfying ???^ = ?,-??;, i < j. Write ?* = ? fia ® ??? and define
?;·(?, ? r) = (-l)w ^ /??(?)???> ???[?- l]p.
Now for each ? G ?[?]? let ?? ? I be the set of indices i such that ? ? F(i)p.
It is immediate from the definition that the elements ?^·(?) G (ApL)n-i satisfy
Since ???, is extendable (Lemma 10.7 (iii)) we may find ?? G (ApL)n such that
?,·?? = ?;.(?), i G ??.
Finally, identify <7?(?[?]H(^??/)? with the set of functions ?[?]? ->· (^PL)n,
just as above, and define ? G Cp(A[n]) 0 (-4pL)n by
? (?) = ?? ,?€?[?]?.
It is then immediate that (di 0 9?)? = ??, as desired. ?
proof of Theorem 10.9: The first assertion is Lemma 10.11. The second
follows by applying Proposition 10.5 to the morphisms
0c ¦¦ Cpl —> CPL 0 Apl and ?? : APL —> Cpl 0 .4pL
given by 7 h-> 70I and ? ?-> 10?. Indeed all three simplicial cochain algebras are
extendable (Lemma 10.7 and Lemma 10.12). Moreover, again by the same two
lemmas, for each ? > 0, H((CPL)n) = H((APL)n) = H((CpL®APL)n) = h ¦ 1.
This trivially implies that ?((??)?) and ?((??)?) are isomorphisms. Thus the
hypotheses of A0.5) are indeed satisfied. D
Finally, let L c ? be a sub simplicial set. Then the quasi-isomorphisms of
Theorem 10.9 restrict to quasi-isomorphism
C'(K,L) -^ (CpL®APL)(K,L) ^ APL(K,L).
128 10 Commutative cochain algebras for spaces and simplicial sets
Thus the two short exact sequences
0 _> C*(K,L) —> C*(K) —> C*(L) —> 0
and
0 -» APL(tf,L) —> APL{K) —> yipi(L) -* 0
are connected by a chain (of length two) of quasi-isomorphisms. In particular
the canonical isomorphisms of cohomology define an isomorphism of long exact
cohomology sequences,
Of course ? and L may be replaced here by a topological space X and a subspace
A.
(e) Integration and the de Rham theorem.
If ? is a simplicial set then Apl(K) is a commutative cochain algebra and
C*(K) is not, which explains why in Theorem 10.9 we need to connect them
by the chain of quasi-isomorphisms C*(K) -=> · «=- Apl(K). In this topic we
show how 'integration' provides a natural direct quasi-isomorphism of cochain
complexes
The quasi-isomorphism § was first constructed in the 1930's in the context of
smooth manifolds and ordinary differential forms. In that setting the fact that
§ commutes with the differentials is precisely Stokes' theorem: ?? = ?,
•/? Jqa
and the fact .that H{§) is an isomorphism was conjectured by E. Cartan and
proved by de Rham [43].
Now recall the constructions in §10(c) and (d) of the simplicial cochain algebras
Apl and Cpl- We shall construct a quasi-isomorphism of simplicial cochain com-
plexes, § : Apl —> Cpl (as defined in §10(a)) and set §K = §{K) : Apl(K) —>·
Cpl(K) = C*(K). There are a number of calculations (mostly omitted) which
are usually easy consequences of the classical integration by parts formula,
¦<???4)
To define § : Api —> Cpl we need to define a sequence of cochain complex
morphisms
" " ? -»<rnr)„ = C*(A[n]) , n>0,
Sullivan Models 129
compatible with the face and degeneracy maps. Recall that (-4pz,)n = ?(??...., tn,
dtu...,dtn). Define a linear map Jn : {AplI^ —> -fe by setting
f / ? ? ? \ ?1 ?1'*1
? ( ? ?1 ?,2 · · · i„" dt ? ? · · · ? dtn 1 = / dti dt-2 ¦ ¦ ¦
? \ ' Jo Jo
? ] \^---tk^dtn
Jo
Now (cf. §10(a)) let ? = (eio ¦ --eik) be a fc-simplex of ?"; i.e. 0 < i0 < h <
¦ ' ' < h < ?. Then ? determines the morphism ?* : (Apl)ti —> (-'Ipl)/: given
by Us >-> tj,l <j <k, and tm ·-> 0 if ?? ^ iOl ·.., ?··
Define fn : (APL)n —> (Cpi)n by setting
(Note that if ? is degenerate then ?*? = 0 and so [§n ?) (?) = 0, as required.)
Theorem 10.15
(i) ? = {<fn} : Apl —> Cpl is a quasi-isomorphism of simplicial cochain
complexes.
(ii) For each simplicial set ? and topological space X the linear maps §K =
§{K) and §? — §{X) are natural quasi-isomorphisms
§K:APL{K)-^C*{K) and §? : APL(X) -> C*(X)
of cochain complexes.
proof: A quasi-isomorphism of extendable simplicial cochain complexes in-
duces a quasi-isomorphism of cochain complexes when applied to any simplicial
set (Proposition 10.5). Since C*(—) = Cpl(-) and since Apl and Cpl are ex-
tendable (Lemmas 10.11, 10.7 and 10.12) the assertion (ii) of the theorem follows
from assertion (i).
To prove (i) we show first that each § commutes with the differentials, which
is essentially Stokes1 theorem. For this it is sufficient to verify that
]T(-ir+i+1 / ??? , ? G (APLrn-1 ,
,·_? Jn-1
130 10 Commutative cochain algebras for spaces and simplicial sets
where ?{ = (e0 ¦ · -ej-iej+i ¦¦¦en) : ??"^—* ?". By linearity it is enough to
consider ? of the form i* ' · · · i*ndtx ? · · · dtj ¦ ¦ ¦ ? dtn (dtj is deleted). But in this
case the formula is a straightforward calculation via A0.14).
Next the compatibility of the §n with the face and degeneracy morphisms
follows from the equation
{§rAPL{a)$) (r) = (-1)^ [?*?*? = (-1)!?^ [(??)*?
J k Jk
valid for all simplicial maps Ak -^ ?? -^» ?" and all ? G (-4pl)? .
Finally, to show that the §n are quasi-isomorphisms notice that jfn(l) = 1 and
recall that H ((APL)n) = Jk = H ((CPL)n), ? > 0 (Lemmas 10.7 and 10.12). D
Remark Multiplication defines a morphism
multn : (CPL)n 0 (CPL)n —> (CPL)n
of cochain complexes (but not of algebras), and so mult = {multn} is a morphism
of simplicial cochain complexes. Thus we have the commutative diagram
Cpl
\ _ /
,? = mult ? (id <g><f) ,
of simplicial cochain complexes, which translates to the obvious diagram when
applied to any simplicial set or topological space. D
Exercises
1. Prove that Apl(*) = Ik.
2. Give an explicit formula for each <9,· : (APL)i -> (APLH = Ik as defined in
(c).
3. Let ? be a simplicial set, and A, B simplicial cochain algebras. Prove that if
H(A) = Ik = H(B) and if A and ? are extendable then there exists a sequence
of quasi-isomorphisms A(K) -+ A(K) 0 B(K) <- B(K).
4. Prove that A = {(An,dn)}n>0 ,9; ,sj is a simplicial algebra where An =
?(??,??,...,??) 0A(?/o,2/i,..-,2/n) with dx{ = y{ and
ii k <i ( xk if k < i
if ?; = 2 and Sj(xfc) = < xkXk+i ii k = i
if k >i I xk+l if k > i
11 Smooth Differential Forms
In this section the ground ring is M.
The construction Api{—;lk) of polynomial differential forms in §10 was sug-
gested by the classical cochain algebra Adr(M) of smooth differential forms on
a smooth manifold M. In this section we review the construction of Adr{M)
and establish a chain of quasi-isomorphisms
of commutative cochain algebras. This implies (§12) that Adr(M) and Apl(M; M
have the same minimal Sullivan algebras and hence that many rational homo-
topy invariants (e.g. dim7rfc(M) ®Q, k > 2 and the rational LS category of ?/)
can be computed directly from Adr(M).
This section is organized into the following topics:
(a) Smooth manifolds.
(b) Smooth differential forms.
(c) Smooth singular simplices.
(d) The weak equivalence Adr(M) S APl(M;R).
(a) Smooth manifolds.
A topological ?-manifold M is a second countable metrizeable topological
space in which each point has an open neighbourhood homeomorphic to an open
subset of M.n. Recall that a map from an open subset of Mn to W1 is infinitely
differentiable or smooth if all its partial derivatives of all orders exist. A smooth
atlas (Ua,ua)aex for M is a covering of M by open sets Ua together with
homeomorphisms ua of Ua onto an open subset of W1, and such that for each
?,? : UaU?1 : U?{Ua ? Up) -^ ua(Ua ? U?) is smooth. Each (Ua,ua) is called
a chart in the atlas. A smooth ?-manifold is a topological ?-manifold together
with a smooth atlas.
Example 1 W1.
The identity map of W1 identifies W1 as a smooth manifold. D
Example 2 Open subsets.
Suppose (Ua,ua)a?Z is a smooth atlas for a smooth manifold M. If ? is an
open subset of M then (ua ? ?, ua L n) is a smooth atlas for 0, and thus
identities ? as a smooth manifold. D
132 11 Smooth Differential Forms
Example 3 Products.
If (Ua,ua)a?j and (Vp,V?)?ej are respectively smooth atlases for a smooth
?-manifold M and a smooth fc-manifold ? then (Ua x V,3, ,ua ? ? ?) identifies
? ? ? as a smooth n + k manifold. ?
Suppose (Ua,Ua)a?i and {Ve,V?)?&j are respectively smooth atlases for a
smooth ?-manifold ?/ and a smooth fc-manifold TV. A smooth map f : M —> ?
is a continuous map such that each V?fu^1 : uQ (Ua Of (V?)) —> ve(V?) is
smooth. The composite of smooth maps is smooth, and a diffeomorphism is a
smooth map admitting a smooth inverse. Finally a smooth function is a smooth
map M —> M and pointwise addition and multiplication makes these into a
commutative algebra, C°°(M). For example, the smooth functions ula : Ua —> M
defined by uQ = (u^,... .u^) are called local coordinates in M. As another
example, if ? G <9open c M then there is always a smooth function f : M —>
[0,1] which vanishes outside O. and is identically 1 in a neighbourhood of a; [69,
Prop. VIII, sec.1.8]. Such a function is called a localizing function for ? G O.
Two smooth maps f.g : M —> ? are smoothly homotopic if there is a smooth
map F : ? ? ? —> ? such that F(-, 0) = / and F(-, 1) = g. For example, the
identity map of Kn is smoothly homotopic to the constant map Rn —^ {0} [69,
Example 1, sec. 1.10].
(b) Smooth differential forms.
Let M be a smooth ?-manifold with smooth atlas {(Ua,ua)}. Every smooth
map u : M —> ? determines the morphism C°°(u) : C°°(M) <— C°°(N) given
Definition The tangent space TXM at ? ? M consists of the linear maps
? : C°°(M) —> M such that ?(fg) = ^fg{x) + /(?)??. If u : M —> ? is smooth
then Txu : TXM —> TUXN is the linear map given by (?????(/) = ? (C°°(u)/).
If ? is an open subset of W1 then a canonical isomorphism Rn -^U TXO is
given by h ?—? ?/?, where
= lim^
[69, Prop. 1, sec. 3.3]. This isomorphism maps the standard basis of W1 to the
partial derivatives d/dxl.
Moreover, if i : ? —> M is the inclusion of an open subset then each Txi
is an isomorphism (use localizing functions) and, finally, if F : M —> ? is a
diffeomorphism then each TXF is an isomorphism. Thus altogether we obtain
isomorphisms
txm Atxuq ATUaX{uaua) Am71, xeuQ . A1.1)
The basis of TXM corresponding to the standard basis of W1 will be denoted by
¦?-, for the obvious reason.
Sullivan Models 133
Now suppose / G C°°(M). The gradient of f at ? e M is the linear map
(df)x : TXM —> M given by ?/?(?) = ?f. The gradients (dul)x,..., (du?)x are
the basis of (???)* = Horn (Tx il/. M) dual to the the basis ^fr, and
dfx = Y,^-{x){du>a)x. A1.2)
A differential p-form on il/ is a family ? = {?? G \p(TxM)s}xeM. For
? € Ua we have
and ? is called a smooth differential p-form if the A,·,...,·,, are smooth functions
in Ua. The vector space of smooth differential p-forms on M is denoted by
APDR{M) and pointwise wedge multiplication makes Adr(M) = {ApDR(M)}p>0
into a commutative graded algebra. Note that A°DR(M) = C°°(M) and that
Adr(M) vanishes in degrees > n.
Formula A1.2) shows that df = {{df)x} is a smooth 1-form on il/; it is called
the gradient of /. Extend d to AppR{M), ? > 1 as follows. For ? G APDR(M)
write ? = ? ??,...???/??^ ? · · · ? du<? in Ua and define c/? G .4^ (il/) by
c/? = EcfAij...^ ? du^1 ? · · · ? du^ .
It is straightforward to verify that this definition is independent of the choice
of chart, and that d is a derivation of degree 1 in Adr(M)· Moreover, for
/ G C°°(M) we have cPf = d fe$rdula) = ? ? ?L d< ? du{a = 0 because of
the symmetry of partial derivatives and the skew symmetry of the wedge product.
It follows that d2 — 0 in Adr(M) and so Adr(M) becomes a commutative
cochain algebra.
Definition The cohomology algebra ? (Aor(M)) is called the de Rham co-
homology of il/.
Finally, if F : M —> ? is smooth then it is easy to see that C°° (F) extends
to a unique morphism Adr(F) : Adr(M) <— Adr(N) of cochain algebras (use
localizing functions).
(c) Smooth singular simplices.
Recall that the standard simplex ?* C Rk+1 consists of the points ? =
(to,...,tk) such that each ?; > 0 and Eti = 1. A smooth singular k-simplex
m a smooth manifold M is a continuous map ? : Ak —> M that extends to a
smooth map from some open neighbourhood of Ak in Rk+1. The smooth singu-
lar simplices form a sub simplicial set. 5^ (il/), of the simplicial set 5* (il/) of all
angular simplices (§10(a)). The correspond cochain algebra (§10(d)) is denoted
134 11 Smooth Differential Forms
by C^(M) = C* Ef (Af);R). It F : M —* ? is smooth then S*(F) and C*(F)
restrict (factor) to morphisms S^°(F) and C^(F).
Next, we introduce smooth differential forms on the standard simplices, ?*,
and the simplicial cochain algebra Adr referred to brief!)' in §10(c). Note that
k
the condition J] U = 1 defines an affine fc-space Fk C R+1 which is, in particular
?
a smooth fc-manifold.
Set Tx(Ak) = Tx(Fk), ? € Ak. Then a smooth differential p-form on ?* is
a family ? = {?? G ApTx(Aky} ? ^k that extends to a smooth differential p~
form on Fk ; as with smooth manifolds we denote the space of smooth differential
p-forms on ?* by ^^(?*). It is easy to see that the kernel of AjjR(Fk) —>
Ar>ft(Ak) is preserved by d, so that A?>R(Ak) inherits a differential.
Now let A; and Qj denote the face inclusions and degeneracies for the sim-
plices Ak, as defined in §4(a). Then set {AoR)k = ????(??') and observe that
{(ADR)n}n>Oi ADR(\i), Adr(bj) is a simplicial cochain algebra, which we de-
note simply"by Ajjr. As observed in Remark 1 of §10(c), there is a natural
inclusion
APL( :R)
of simplicial cochain algebras. Moreover, straightforward calculations establish
Lemma 11.3
(i) (ADR)o = M.
(ii) H((ADR)n) = R, n> 0.
(Hi) Each APDR is extendable.
(d) The weak equivalence ADR{M) ~ APL(M;R).
Let M be a smooth manifold, and recall that S^°(M) denotes the simplicial
set of smooth singular simplices. In particular, we may apply the construction
of §10(b) with the simplicial cochain algebra Adr to obtain the cochain algebra
Adr E~(M)) whose elements are the families ? = {?? 6 -4??(?'?'}?€5??(?)
compatible with the face and degeneracy operators.
On the other hand, any ? ? S^°(Ad) determines the morphism Adr{ct) ¦
Adr(M) —> ???(?|?|). Thus a natural morphism
<*M : Adr(M) -> ADR (S?
is defined by aM : ? "—> {^?»?(?)?}?€5?(?).
Moreover, recall that APL(M;R) = ~APL(St(M);R). Thus the inclusion
SZ°{M) —-)· S,{M) and APL(;R) —> ADR define natural morphisms
Sullivan Models 135
Theorem 11.4 The morphisms olm,&m and 1m are all quasi-isomorphisms.
In particular, Adr(M) is weakly equivalent to Apl(M;R).
For the proof of Theorem 11.4 we consider first an arbitrary natural trans-
formation ? : A —> ? between functors from smooth ?-manifolds to cochain
algebras. We suppose, however, that
(i) H(A(mn)) = m = H(B(mn)).
(ii) If U,V are open in M and ??,?? and ???? are quasi-isomorphisms, then
so is
(iii) If ? = UOi is the disjoint union of open sets Oi then ?? = ? ^O; :
Lemma 11.5 With the hypotheses above, ? ? is a quasi-isomorphism for all
smooth ?-manifolds M.
proof: An i-basis for M is a family of open sets V\ c M, closed under finite
intersection, and such that any open subset of M is the union of some of the
V\. Given such an i-basis it is possible to write M = ? U W where ? =
i
W = U Wj and each O, and Wj is a finite union of elements of the i-basis. If
j
each ??? is a quasi-isomorphism it follows by induction on ? that each ??? ?···???
is a quasi-isomorphism and hence that ?0, ??? and 9onw are too. Thus in this
case ?? is a quasi-isomorphism.
Now suppose U is open in Mn. A standard cube in Mn is an open set V of the
form (oi,6i) x ··· x (an,bn) and each ?? is a quasi-isomorphism by (i) above.
But the standard cubes contained in U are an i-basis for U and so ?? is a quasi-
isomorphism. But the open subsets of M diffeomorphic to open subsets of W1
are an i-basis for M and so ? ? is a quasi-isomorphism. D
proof of Theorem 11.4: (i) Pm is a quasi-isomorphism. Lemmas 10 and 11.4
assert that Apr,(—;R) —> A^r is a quasi-isomorphism of extendable simplicial
cochain algebras. Thus Proposition 10.5 asserts that ?iu is a quasi-isomorphism.
(ii) im is a quasi-isomorphism. The inclusion S^°(M) —> S*(M) induces
Qm ¦ C*{M) —> C^(M), and Theorem 10.9 identifies ?{??) and ?(??).
On the other hand if /, g : M —> ? are smoothly homotopic then the ho-
motopy C»(/) — C*(g) = dh + hd defined in §4(a) restricts to a homotopy be-
tween Cf (/) and C^°(g). Thus since the identity and constant maps in Mn are
smoothly homotopic we conclude that H (C*(Mn)) = R = H (C^(!n)).
Next, if U and V are open subsets of M then the barycentric subdivision ar-
gument [121] that shows that C,(U) + Cr(V) A C,(U U V) also shows that
C?(U) + C?(V) -=? C?(U U V). Thus a long exact homology sequence argu-
ment shows that if C™(U) —> C,(U), Cf (V) —> C,(V) and C?(U ? V) —>
136 11 Smooth Differential Forms
C,(U ? V) are all quasi-isomorphisms then so is C?°(U U V) —> C,{U ? V).
Dually, if 7i/,7v and 71/nV are quasi-isomorphisms then so is 7c/uV·
Finally, if ? = Jj Oi then clearly 70 = ??7?;· Thus Lemma 11.5 asserts that
I i
7?? is a quasi-isomorphism for all M.
(iii) ?·? is a quasi-isomorphism. First note that ? (AorO^11)) = M (clas-
sical Poincare lemma — [69, Example 1, sec 5.5]) while H (ADr E~(Mn))) =
H(C?°(Rn)) = M as we showed in (ii) above. Next observe that if U and V*
are open in M then the difference of restriction morphisms defines a short exact
sequence
0 -> ADR(U UV)^ ADr(U) © ADR(V) -> ADr(U ? V) —> 0
[69, Lemma 1, sec. 5.4]. Similarly we have the short exact sequence
0 -> ADR (S?(U) U S?
and a long exact cohomology sequence argument shows that the composite
ADr(U U V) -> Adr (S?(U U F)) -> ADR (S?(U) U 5,°
is a quasi-isomorphism if ??,?? and aunv are.
Since Proposition 10.5 identifies ? {Adr{K)) with if (C*(K)) for any simpli-
cial set ? and since as in (ii) above ? (C™(U) + C?°(V)) A if (Cf(i/ U V))
it follows that ADR E»°°(i/ U V)) —> AD? E~(?/) U 5~(F)) is also a quasi-
isomorphism. Hence so is ??/??·
Finally, if ? = ]JOi then ao = ???<· Thus Lemma 11.5 states that a m is
i i
a quasi-isomorphism for all M. D
Exercises
1. Let M be a differential manifold with a fixed open cover 11 = {i/o, C/i, ·.·, i/m}.
We denote by jVp the set of sequences / = (io,i\,...,ip) such that 0 < io < ii <
... < ip < m and Ui := t/io ? t/^ ? ... ? Uip ? 0. Define dL : Mp+l -> Afp
by 9/(io,ii,...,ip+i) = (??,?,···,?,··?«?+?) ^d denote by pf : Adr(Ul) ->
the restriction map induced by an inclusion Uk C Ul- We set:
(il), d" : Cp'q(IX) -> Cp'q-
p+i
Prove that (C*(il) ,d! + d") is a cochain algebra with the product defined by:
if ? = (io, h, --,? + ?') € ??+?' , and
r — ?·. ?. 7;„) ? 7^ /' = (i i 4-1 ... i + ')
Sullivan Models 137
Is this product commutative? Prove that if each Ui is contractible in M then
the graded algebra H(C*(ii) ,d! + d") is isomorphic to H*(M; R).
2. Let M be a connected ?-manifold and take a nicely imbedded ?-disk D in
M. Denote by A'(M) the subalgebra of differential forms on M vanishing on
the disk D. Prove that there is a sequence of quasi-isomorphisms
A(M) <^-> A'{M) c^> ADR{M),
where A(M) denotes a connected subalgebra of .4'(il/).
3. Let M and ? be compact connected oriented ?-manifolds. We define the
connected sum of M and JV, ?#??, as follows : take a nicely imbedded n-disk
D in M and in N, remove their interiors, and paste the boundaries together
via an orientation reversing homeomorphism. We use the notation of exercise
2) and denote by um ? A(M) (resp. ?/? ? A(N)) a cocycle representing the
orientation class of M (resp. of N). Prove that there is a quasi-isomorphism:
{[Adr{M) ©r A(N)) ? uM, d) -» ADR(M#N),
with d(u) = um — ?/?· Deduce that the cohomology ring H*(M#N;Ik) is the
direct sum of H*(M: Ik) and H*(N: Ik) with the units and the orientation classes
identified.
12 Sullivan models
In this section the ground ring is an arbitrary field ic of characteristic zero.
Having constructed the functor
Apl ¦ topological spaces ~» commutative cochain algebras
in §10, and the functor Adr: smooth manifolds ~» commutative cochain algebras
in §11, we focus now on the study of commutative cochain algebras themselves.
Here the principal role is played by the Sullivan cochain algebras (or Sullivan
algebras for short) which we introduce now:
Definition A Sullivan algebra is a commutative cochain algebra of the form
(AV,d), where
• V = {Vp}p>i and, as usual, AV denotes the free graded commutative
algebra on V;
oo
• V = U V(k), where V@) C F(l) C ··· is an increasing sequence of
k=0
graded subspaces such that
d = 0 in V@) and d : V(k) —» AV(k - 1), k > 1.
The second condition is called the nilpotence condition on d. It can be restated
as: d preserves each AV(k), and there exist graded subspaces Vf. C V(k) such
that AV(k) = AV(k - 1) <g> AVk, with d:Vk-> AV(k - 1).
Observe that a Sullivan algebra is completely described by the vector space V
and the linear operator, d. By contrast, for a general commutative cochain
algebra (A, dA), the 'non-linear' multiplicative structure of A is also impor-
tant. Nonetheless, if H°(A) = k then we shall show that there always exists
a quasi-isomorphism from a Sullivan algebra to (A,d). This applies in particu-
lar to Apl(X) for X a path connected topological space, since H° (Apl(X)) =
H°(X;k) = lk(d. A0.1)).
Definitions 1 A Sullivan model for a commutative cochain algebra (A, d) is a
quasi-isomorphism
??: (AV,d) —* (A,d)
from a Sullivan algebra (AV, d).
2 If X is a path connected topological space then a Sullivan model
for APL(X),
m: (AV,d) ^> APL(X),
is called a Sullivan model for X.
3 A Sullivan algebra (or model), (AF, d) is called minimal if
imdC A+V -A+V.
Sullivan Models 139
We shall frequently abuse language and refer simply to (AV, d) as the Sullivan
model for (A,d) or for X. If H°(A) = Ik then (A,d) always has a minimal
Sullivan model, and this is uniquely determined up to isomorphism. This will
be shown here in the simply connected case, and in general in §14. In §14 it
will be shown that every Sullivan model (or algebra) is the tensor product of its
unique minimal model with a Sullivan algebra of the form (A(U ? SU), ?), where
? : U -^ OU. Sullivan algebras of this form are called contractible.
Sullivan models for topological spaces X are, among all the commutative mod-
els, the ones that provide the key to unlocking the rational homotopy properties
of X. For example, if (AV,d) is a Sullivan model then (cf. A0.1)), as with any
commutative model,
H(AV,d) ? H*(X;]k).
However, if (AV, d) is minimal there is also a natural isomorphism
provided that X is simply connected and has rational homology of finite type.
(The isomorphism is established in §15, based on a construction in §13.)
Recall further from §10 that if simply connected topological spaces X and Y
have the same rational homotopy type, then Apl(X) and Apl(Y) are weakly
equivalent. Since minimal models are unique up to isomorphism, this implies
that Apl(X) and Apl(Y) have isomorphic minimal models: the isomorphism
class of a minimal model of X is an invariant of its rational homotopy type. In
§17 we shall show that this defines a bijection,
rational homotopy ? ~ j isomorphism classes of ?
types J \ minimal Sullivan algebras over Q J
where on the left we restrict to simply connected spaces with rational homology
of finite type, and on the right to Sullivan algebras (AV, d) with V1 = 0 and each
V* finite dimensional.
Sullivan models also provide good descriptions of continuous maps, and of
the relation of homotopy. Indeed, let A(t, dt) be the free commutative graded
algebra on the basis {t, dt} with deg t = 0, deg dt = 1, and let d be the differential
sending t ?-»· dt. As noted in §10, H (A(t,dt)) = Jk. Define augmentations
??,?? :?(?,?) —> A by ??(?) = 0, ??(?) = 1.
Definition Two morphisms ??,?? : (AV,ci) —> (A,d) from a Sullivan alge-
bra to an arbitrary commutative cochain algebra are homotopic if there is a
morphism
? : (AV, d) —> (.4, d) <g> (A(i, dt), d)
such that (id-Si)$ = ??, i = 0,1. Here ? is called a homotopy from ?0 to ?,
and we write ?? ~ ?-
12 Sullivan models 140
We shall see that homotopy is an equivalence relation. Moreover, suppose
mx : (AV.eQ —> ApL(-X) and my : (ATV,d) —> APL(y) are Sullivan models
defined over Q, and that / : A' —> Y is a continuous map. Then it turns out that
there is a unique homotopy class of morphisms ? : (AW, d) —> (AV, ci) such that
???? ~ ???(/)???: ? is called a Sullivan representative for /. Furthermore,
the homotopy class of ? depends only on the homotopy class of /. If X and Y
are rational spaces (cf. §9) with rational homology of finite type, then we shall
show in §17 that / \—> ? defines a bijection
homotopy classes of ? 2 ? homotopy classes of
maps X—>Y j \ morphisms (A W,d)—> (AV,d)
Finally, and perhaps of most importance, Sullivan algebras and models provide
an effective computational approach to rational homotopy theory. In this and
the next section we shall emphasize that approach, complementing the basic
propositions with a range of examples. The introduction of relative Sullivan
algebras, and the proofs of a number of theorems, follow in §14 and §15.
This section, then, is organized into the following topics:
(a) Sullivan algebras and models: constructions and examples
(b) Homotopy in Sullivan algebras.
(c) Quasi-isomorphisms, Sullivan representatives, uniqueness of minimal mod-
els and formal spaces.
(d) Computational examples.
(e) Differential forms and geometric examples.
(a) Sullivan algebras and models: constructions and examples.
We begin by recalling notation and basic facts associated with free commuta-
tive graded algebras AV — cf. §3(b), Example 6. These will be used without
further reference.
• AV = symmetric algebra (Feven) <g> exterior algebra (Vodd). The subalge-
bras A (V^p), A (V>"),... are denoted AV^, AV>",....
• // {va} or vi, vq, ... is a basis for V we write A({va}) or ?(?? ,vo,...) for
AV.
• A9V is the linear span of elements of the form vi ? · · · ? vq, Vi 6 V.
Elements in AqV have wordlength q.
• AV = 0A'F and we write A^qV = ? A'V and A+V = A^V.
Q i>q
• // V = ? Vx then AV =
Sullivan Models 141
• Any linear map of degree zero from V to a commutative graded algebra A
extends to a unique graded algebra morphism AV —> A.
• Any linear map of degree k (k 6 Z) from V to AV extends to a unique
derivation of degree k in AV.
In particular the differential in a Sullivan algebra (AV, d) decomposes uniquely
as the sum d — do + di + d-i + · · · of derivations di raising the wordlength by i.
The derivation do is called the linear part of d.
Our first step is to show the existence of Sullivan models:
Proposition 12.1 Any commutative cochain algebra (A,d) satisfying
H°(A) = ]k has a Sullivan model
m:(AV,d) -?> (A,d).
proof: We construct this so that V is the direct sum of graded subspaces Vj.,
/fc-l \
k > 0 with d = 0 in Vo and d : Vk —> A 0 V{ . Choose m0 : (AFo,0) —>
\i-0 /
(^4, d) so that
if(mo) : Vo ? ?+(?).
Since H°(A) = k, H(m0) is surjective.
( ( k \ \
Suppose m0 has been extended tom^ : ( ? I 0 Vi I ,d I —> (A,d). Let za
\ \i=o J J
( k \
be cocycles in ? ? Vf such that [za\ is a basis for ker ?(??^). Let V'^+i
\?=? /
be a graded space with basis {va} in 1-1 correspondence with the za, and with
fk+l \
aegva = aegza — 1. Extend d to a derivation in ? I 0 Vj I by setting dva — za.
\i=o J
Since d has odd degree, d'2 is a derivation. Since drva = dza = 0, d2 = 0.
Since H(mk)[za] — 0, mkZa = daa, aa 6 .4. Extend m^ to a graded algebra
A+i \
morphism mt+i : ? I 0 V{ ) —> A by setting mt+it;Q = ??. Then mk+idv^ =
\i=0 /
«mt+ifai and so mt+1d = dm,k+i.
oo
This completes the construction of m : (AV", d) —> (A,d) with V = 0 \'\
and m |y^= m/.. Since m ?? = m0, and H(m0) is surjective, i/(m) is sur-
( k \
jective as well. If H(m)[z] = 0 then, since ? is necessarily in some A 0 V-t ,
\t=o /
= 0. By construction, ? is a coboundary in A ( 0 Vi ). Thus H(m)
\i=0 /
is an isomorphism.
142 12 Sullivan models
We show next by induction on k that 14 is concentrated in degrees > 1. This
is certainly true for k = 0, because Vo = H+(A). Assume it true for V{, i < k.
( k \
Any element in ? ? Vj of degree 1 then has the form
\i=0 /
? = vo + l· Vk, Vi 6 Vi .
( \
Thus if dv = 0 then dvk € d ( ? ? Vi . By construction, this implies
V i=o /
vk = 0- Repeating this argument we find ? = v0 and H(mk)[vo] =
i/(mo)[i>o] ^ 0, unless v0 = 0. Thus kerH{rrik) vanishes in degree 1; i.e., it
is concentrated in degrees > 2. It follows that \\+\ is concentrated in degrees
> 1.
Finally, the nilpotence condition on d is built into the construction. ?
Example 1 The spheres, Sk.
Recall that in §4(c) we defined the fundamental class [Sk] € Hk{Sk;Z). This
determines a unique class ? € Hk (ApL(Sk)) such that {?, [Sk]) = 1. and 1, ?
is a basis for ? (Api(Sk)). Let ? be a representing cocycle for ?.
Now if k is odd then a minimal Sullivan model for Sk is given by
m : (?(?),?) A APL(Sk), Zj?
Indeed, since k is odd, 1 and e are a basis for the exterior algebra A(e).
Suppose, on the other hand, that k is even. We may still define m : (A(e), 0) —>
ApL{Sk) by: dege = k, me = ?. But now, because dege is even, A(e) has as
basis 1, e, e2, e3,... and this morphism is not a quasi-isomorphism. However, ?2
is certainly a coboundary. Write ?2 = dty and extend m to
m:(A(e,e'),eQ —> APL(Sk)
by setting dege' = 2k — 1. de' — e~ and me' = ?. A simple computation shows
that l,e represents a basis of H (A(e,e'),d). Thus this is a minimal model for
5*.
Finally, observe that quasi-isomorphisms
(Ae,0) —» (H'(Sk),0) , k odd and (A(e,e'),ci) -=> (H*(Sk),0), k even
are given by e h> ?, e'i-> 0.
Example 2 Products of topological spaces.
Suppose ??? : (AV, d) —> Apl(X) and ??? : (AW,d) —> APi{Y) are Sullivan
models for path connected topological spaces X and Y. Assume further that the
rational homology of one of these spaces has finite type. Let px : ? ? ? -*· ?
and ?? : ? ? y -» ? be the projections. Then APL(px) ¦ APl(py)
Apl(Y) —> APl(X ? ?) is a quasi-isomorphism of cochain algebras.
Sullivan Models 143
In fact, ???(??) ¦ ???(??) is clearly a morphism of graded vector spaces
commuting with the differentials. It is a morphism of algebras because Api (X x
Y) is commutative. To see that it is a quasi-isomorphism use Corollary 10.10 to
identify the induced map of cohomology with the map
H*(X; k) ® H*(Y; k) —> H*(X ? Y; k)
given by ? ®? >-» H*(px)aU?*(??)?. But Propo«ition 5.3(ii) asserts that this
map is an isomorphism.
Since Api(px) ¦ Api(pY) is a quasi-isomorphism so is
???-???: (AV, d) ® (AW, d) A APL(X ? ?) ,
where (m ? ¦ ???)(? ® b) = ??^(??)???? ¦ ApL(pY)b. This exhibits (AV,d) ®
(AW, d) as a Sullivan model for ? ? F. Observe that if (AV, d) and (AW, d) are
minimal models then so is their tensor product. ?
Example 3 ?-spaces have minimal Sullivan models of the form (AV. 0).
An ?-space is a based topological space (X, *) together with a continuous
map ? : X x X —> X such that the self maps ? \-? ?(?, *) and ? t-t ?(*, ?) of X
are homotopic to the identity. We establish a result of Hopf:
• If X is a path connected ?-space such that H*(X; k) has finite type then
H*(X;k) is a free commutative graded algebra.
To see this, observe first that because H,(X; Ik) has finite type, ?*(?) can be
identified as a morphism of graded algebras,
?*(?) : H'(X; k) —> H*{X; k) ® H*(X; k).
Moreover, the conditions ?(?, *) ~ id, ?(*, x) ~ id imply that for h 6 H+(X; k),
?*(?)? = /?®1 + ? + 1®/?, some ? 6 H+(X; k) ® H+(X; k).
Now choose a graded space V C H+(X; k) so that H+(X: k) = V®H+(X; k) ¦
H+(X; k). The inclusion extends to a morphism ? : AV —> H*(X; k) of graded
algebras and an obvious induction on degree shows that ? is surjective.
Suppose by induction that ? is injective in AV<n, and let ? : H*(X; k) —>
H*(X; k)lip(AV<n) be the (linear) quotient map. The general element in AV-n
can be written as a finite sum w = J2 v^v!;2 ¦ ¦ -^"?^^...^, where the
fcl kr.
ati—fcr 6 y\.V<n, the Vi are linearly independent elements in V" and ki = 1 or 0
if ? is odd. Then the component of (? ® ??)?*(?)??? in (Im ?)" ® H*(X; k) is
given by
,klt...,kr
144 12 Sullivan models
By construction, the nvt are linearly independent. Hence if ??? = 0 then
<p(llkiVi1---vt1-1---v$*ak1...kr) = 0· By induction on degw, ?^??1 ¦ ¦ ¦
?*' · · •Urrafe1...tr = 0 for each i. Thus each a^...^ — 0 unless ki — ¦ ¦ · =
fcr = 0. Then w 6 AV<n and ??? = 0, whence tu = 0 by induction. It follows
that ? is injective in AV~n. Thus, by induction, ? is injective and the proof of
Hopfs result is complete:
<p:AV-=> H'(X;k).
Finally; let ui) 6 Apl{X) be cocycles representing the cohomology classes Uj.
The correspondence vi t-t iui defines a linear map V —> Apl(X) which extends
to a unique morphism 7?i : (AV.O) —> Api(X). Since ? is an isomorphism it
follows that m is a quasi-isomorphism:
m:(AV,0) -
is a minimal Sullivan model for the ii-space X. ?
Example 4 A cochain algebra (AV, d) that is not a Sullivan algebra.
Consider the cochain algebra (A,d) = (A(vi,v2.,v3),d), deguj = 1, with dvx =
v2v3, dv2 = v3vi, and dv% = vivo- Here (A,d) is not a Sullivan algebra. (If it
were, it would have to have a cocycle of degree 1). The cocycles 1 and v^vov^
represent a basis for H (A), and so it has a minimal model
m : (?(??),?) -% (AV,d), degm = 3, m(w) = viv-2v3. ?
Example 5 In contrast with Example 4> o.ny cochain algebra of the form
(A,d) = (AV,d), V = V^2, imd CA+V-A+V
is automatically a minimal Sullivan algebra.
Indeed, in this case necessarily (A+V ¦ A+V)k+1 C AV-11'1 and so for degree
reasons alone,
d: Vk —> AV-k~l.
This exhibits (AV, d) as a Sullivan algebra. ?
Again, suppose (A,d) is a commutative cochain algebra. In §14 we shall show
that if H°(A) = Jk then (A,d) has a (unique) minimal Sullivan model; this
follows from a more general result about relative Sullivan algebras. However,
if also H1 (A) = 0 then there is a simple inductive construction for a minimal
model. We carry this out here, and prove uniqueness in this context in (c).
Thus suppose given (A,d) with H°(A) = k and Hl(A) = 0.
• Choose m2 : (AV2,0) —> (A,d) so that H'2(m2) : V2 A H2(A). Note
that Hl(m2) is an isomorphism because HX(A) — 0 and that H3(m2) is
injective because (AV2K = 0.
Sullivan Models 145
• Supposing that mk : (AV-k,d) —> (A,d) is constructed, we extend to
mk+\ : (AV'-fc+1,d) —> (A,d) by the following procedure.
Choose cocycles aa ? Ak+1 and z? € (Al''-fc)H2 so that
Hk+1 (A) = Im Hk+1 (mk) ? 0 A- · [??] and ker Hk+2{mk) = 01· [zd] .
In particular, mkz? — db?, some 6jj G A.
Let Ft+1 be a vector space (in degree k + 1) with basis {v'Q,v'?} in 1-1 cor-
respondence with the elements {aa}, {z?}. Write AV'^+1 = AV"^fc ® AVk+l.
Extend d and mj, respectively, to a derivation in AF-t+1 and to a morphism
mjfc+i ·' AV-fc+1 —> A of graded algebras, by setting
dv'a = ?,, di$ = ? ? and m^ = aa, ???'? = 6/j.
Since d has degree 1. d2 is a derivation. By construction, dr = 0 in V"fc+1 and
in AV-fc. Thus d2 = 0. In the same way m^+id = dmk+i in Ffc+1 and AV-k,
and so
.Proposition 12.2 Suppose (A,d) is a commutative cochain algebra such that
H°(A) = k and H^A) = 0. Then
(i) The morphism m : (AV, d) —> (A, d) constructed above is a viinimal Sulli-
van model.
(ii) Ifris the least integer greater than zero such that Hr(A) ? 0, then V1 = 0,
1 < i < r and
Hr(m) : Vr A Hr{A).
(Hi) If dim Hk (A) < cc; k > 1, then dim Vk <oo, k>l.
proof: (i) Since d : Vk+l —> AV'-fc, this exhibits (AV,d) as a Sullivan alge-
bra. More, (AV<k)k+2 c A+V^k-A+V^k, and so (AV, d) is minimal. It remains
to show m is a quasi-isomorphism, and this follows at once from the assertion,
iri, \ - ? an isomorphism for i < k , ^ _ .,_ „.
Hl{mk) is { . . f . - ,k>2, A2.3)
v ' \ mjective for ? = A; + 1 - v '
which we prove by induction on k.
For k = 2 A2.3) was observed at the start of the construction above. Suppose
A2.3) holds for some k. Since mt+i extends mk. Im H(mk) C Im H(mk+\).
Thus iP(mfc+1) is surjective for ? < k by induction and surjective for i = k + 1
by construction.
To show Hl(mk+i) is injective for i < k + 2 let [2] be a cohomology class in
kertfl(mfc+1), some ? < k + 2. We have to show [z] = 0. If deg[^] < A: or if
146 12 Sullivan models
deg[z] = k + 2 then ? 6 AF-fc and [?] 6 keriP(mfc)- Thus [?] = 0 by induction
if deg[z] < k and by construction if deg[z] = k + 2.
Suppose deg[2] = fc + 1. Then ? = ????'? + ??0?0' + ??, some u> 6 AV^k, where
we use the notation from the construction above. Since dz = 0, ????? = —dw
and ? ?/3 [2/3] = 0 in H(AV~k). By construction, this implies that each ?/3 = 0.
But then dw = 0 and ???[??] = Hk+1(mk)[w\. Again by construction, each
Xa = 0. Hence ? = w and so [z] 6 ker Hk+l(mk). By induction, [z] = 0.
(ii) This is immediate from the construction.
(Hi) Since V2 = ii(yi), it is finite dimensional. Suppose by in-
duction that V{ is finite dimensional, i < k. Then AV-k has finite type and,
in particular, keriifc+2(mt) is finite dimensional. Since also Hk+1(A) is finite
dimensional it is immediate from the construction that dimF*-'+1 < 00. ?
Corollary Let X be a simply connected topological space such that each Hi(X;Q)
is finite dimensional. Then X has a minimal Sullivan model
such that V = {V1}i>o and eac^ V1 ™ finite dimensional.
proof: The Hurewicz theorem 4.19 shows that Hx{X;lk) = 0, and Hk{X;Jk)
is the dual of the finite dimensional vector space Hk(X',Q) ®q 1; (cf. §3(e)
and Proposition 5.3). Thus H° (APL(X)) = A, H1 (APL(X)) = 0 and each
Hk (Apl(X)) is finite dimensional. Now apply Proposition 12.2. ?
Example 6 Simply connected topological spaces X with finite dimensional ho-
mology admit finite dimensional commutative models.
Suppose X is a simply connected topological space such that H*(X; Q) is finite
dimensional (e.g. a simply connected finite CW complex — cf. Theorem 4.18).
Then X has a minimal model
in which V = {Vl}i>2 and each V* is finite dimensional. In general, AV will not
be finite dimensional, as is already shown by the even spheres 52r (cf. Example
1)·
There is, however, ? '?on-free' finite dimensional commutative model for X,
constructed as follows. Put
?? = max{i | Hl(X; A~) ?- 0}.
Write (AV)nx = ? ? (Imd)"-* ? C, where H © (lmd)nx = {kerd)nx; thus
H A Hn*{AV,d) a Hn*{X;]k). Choose a graded subspace / c (AV,d) so
that
/* = 0, Ar < nx -
rk _
an
Sullivan Models 147
Since V = {^/z}f>9> I is an ideal. It is immediate from the construction that
/ is preserved by d and that H(I,d) = 0 (because Hl(AV,d) = 0, i > ??). Thus
the quotient map
V:(AV,d)^((AV)/I,d)
is a quasi-isomorphism, and so ((AV)/I.d) is a finite-dimensional commutative
model for A'.
Note that (AV)/I vanishes in degrees k > nx and that [(AV)/I]nx =
Example 7 The minimal Sullivan algebra (A(a,b,x,y,z),d), where
da = db — 0, dx = a~, dy = ab, dz = b2
and deg ? = deg 6 = 2 and deg ? = deg y = deg ? — 3.
Here, the cohomology algebra ? has as basis
1, a=[o], ? = [b], ?-= [ay — bx], S = [by — az], ? = [aby — b2x\.
Note that ?? = ? = ?j, and that all other products of basis elements in H+ are
zero.
We can now use the procedure above to construct a minimal model for the
cochain algebra (H,0). This will have the form m : (AV, d) -=+ (H,0), beginning
with
V*2 : v\ dv\ = 0 mv\ = a
vo dv2 = 0 mi'2 = ?
V3 : u\ du\ = v'l mui = 0
U3 duz = v\ muz = 0
Note that necessarily
m(v\U2 — V2U1) = 0 = m(v2U2 — V1U3).
Thus we need to add
yA '¦ x\ dx\ — v\Uo — V2U1 mx\ = 0
Xo dXo = V0U0 ~ V\U3 ???2 = 0,
and
V5 : yi . dyi = 0 myi = 7
2/2 ?/2 = 0 myo = ?.
The process turns out (but we can not yet prove this) to continue without end.
Observe that this provides two distinct minimal Sullivan algebras with the
same cohomology algebra- D
148 12 Sullivan models
(b) Homotopy in Sullivan algebras.
The results in this topic flow from two basic observations. First, consider a
diagram of commutative cochain algebra morphisms
(A,d)
~ ? ?
0W,d) >{C,d)
til
in which ? is a surjective quasi-isomorphism, and (AV,d) is a Sullivan algebra.
Lemma 12.4 (Lifting lemma) There is a morphism ? : (AV, d) —> (A, d)
such that ?? = ? (? is a lift of ? through ?).
proof: We may suppose V is the increasing union of graded subspaces V(k),
k>0 such that V(k) = V(k - 1) ? Vk and d : Vk —> AV(k - 1). Assume ? is
constructed in V(k — 1) and let va be a basis of Vk- Then ???? is defined and
d(ipdva) = ?(?2??) = 0. Furthermore,
????? = ???? — ????.
Since ? is a surjective quasi-isomorphism we can find aa G .4 so that daa = ipdva
and ??? = ???. Extend ? by setting ??? = ??. ?
Second, with a graded vector space U = {Ul}i>0 associate the commutative
cochain algebra (E(U),6), defined by
E(U) = A(U © 6U) and <5 : U A 5U.
It is augmented by ? : (E(U),5) —> it, where e(U) = 0- Note that if U =
{Uz}i>i then this is a Sullivan algebra. Cochain algebras of this form are called
contractible. We recall a remark already made in §12:
Lemma 12.5 ? : (E(U),S) —> A· is a quasi-isomorphism; i.e.
H(E(U),6) = Jfc.
proof: Let {ua} be a basis for U. A direct calculation (using chark = 0 if
degua is even) shows that ? (A(ua,dua)) = Jc. But E(U) = (g) A(ua, oua) and,
a
since h is a field, homology commutes with tensor products (Proposition 3.3).?
As a direct consequence of this lemma we obtain:
The surjective trick: // (A, d) is any commutative cochain algebra, then the
iAontiht of A extends uniquely to a surjective morphism ? : (?(?),?) —> (A,d).
Sullivan Models
149
Thus any morphism ? : (?, d)
as
(B,d)
in which the inclusion ? : b
tive.
(A, d) of commutative cochain algebras factors
b <g) 1 is a quasi-isomorphism and ? · ? is surjec-
Recall that A(i, dt) denotes the special case of (E(U),6) with U = ht and with
degi = 0. It is precisely the cochain algebra (???)?, of polynomial differential
forms on the standard 1-simplex (§10 (c)). The augmentations ?0, ?i : A(i, dt) —>
Ic, co(i) = 0, ci(i) = 1, correspond to the inclusions of the endpoints.
As defined in the introduction to this section, two morphisms ??, ?? : (A V, d) —i
(.4, d) from a Sullivan algebra are homotopic if there is a morphism
? : (?? d) —> (A, d) ® ?(?, dt)
such that (??·??)? = ??, i = 0,1. Given that Api reverses arrows, and that
A(t,dt) is the algebra of polynomial differential forms on the standard 1-simplex.
this is the obvious analogue of a topological homotopy X <— ? ? /.
In fact, Apl 'preserves homotopy' in the following sense. Suppose given con-
tinuous maps /o,/i : X -> Y and a morphism ip : (AV.d) —> Api(Yr) from a
Sullivan algebra (AV.d).
Proposition 12.6
(AV,d) -> APL(X).
If f0 ~ fx : X -> Y then APL(f0)ip ~ APL{fx)yj
proof: Identify A(i,dt) as a subcochain algebra of Apl(I), by mapping t t->
u e A°PL(I), where u restricts to 0 at {0} and to 1 at {1}. Denote by j0, j\ :
X —> X x / the inclusions at the endpoints and by px : ? ? I —> X and
?1 : X y. I —> I the projections. Then
APL(X)®A(t,dt)
(id -??,?? ·??)
APL(px)APL(p') APL{X) X ApL(X)
(AplUo),Apl(Ji))
Apl{X x /)
is a commutative diagram of cochain algebra morphisms.
Since H (ApL(px)) = H*(px;]k) is an isomorphism, Apl(px) · APL(pJ) is a
quasi-isomorphism. We now 'make it surjective'. Let U C APL (? ? /) be the
kernel of (ApL(j0),Apl(Ji)). The inclusion of U extends to a unique cochain
algebra morphism
150 12 Sullivan models
Extend the diagram above to the commutative diagram
APL(X)®A(t,dt)®(E(U),S)
(id ·??·?,»<?·??·?)
APL{X) ? APL(X)
AFL(px)ApL(p')g
ApL(XxI)
Here ^4??,(?? )·???,(??)'9 is, obviously, surjective, and it follows from Lemma 12.5
that it is a quasi-isomorphism too.
Let ? : X x / —> Y be a homotopy from /o to f\. Use Lemma 12.4 to lift
???(?)? : (AV,d) —> Apl{X ? /) through the surjective quasi-isomorphism
Apl(pX) ¦ Api{p!) ¦ Q. This produces a morphism
? : (AV, d) —> Api(X) <g> A(t, dt) ® E(i/).
Then set ? = (id <S> id <g> ?)?; it is the desired homotopy from ApL(fo)^ to
As with homotopy of continuous maps, we have
Proposition 12.7 Homotopy is an equivalence relation in the set of morphisms
? : (AV, d) —> (A,d) from a Sullivan algebra.
proof: Refiexivity is obvious: A homotopy ? : (AV, d) —> (A,d)<S>A(t,dt) from
? to ? is given by ?(?) = ?? <g> 1. Symmetry is also easy: the automorphism
t t-> 1 — t of ?(?,???) interchanges ?0 and ??. For transitivity, suppose
? : (AV,cO —> (A,d) <8> ?^,??) and ? : (AV.cQ —> (A,d) ® A(t2,dt2)
are homotopics from ?? to ?\ and from ?? to ??. Then ? and ? define a
morphism
(?,?) :(AV,d) —> [(A,d)®A(t1,dt1)]xA[(A,d)®A(t2,dt2)]
= (?,?)®[?(^,??) ???(?2,?2)],
where the second fibre product is with respect to the augmentations ?? : ?(??, dt\)
A-, ??(??) = 1, and ?0 : A(t2,dU) —> k, ?0(??) = 0.
Now consider the morphism,
Mp'>·> = ^v> ? y,^^ > A(tudt!) xikA(t2,dt2)
Sullivan Models 151
given by t0 h-> A-??,1-?2), ?? ^ (<i,0), t2 *-* @,i2). Using Proposition 10.4(i)
we may identify it with restriction
APL(A[2])-+APL(L),
where L C ?[2] is the subsimplicial set with non-degenerate simplices (e0), (ei),
(e2), (eo,ei) and (??,??). Schematically L is given by the solid lines in the
diagram
Since Apl is extendable (Lemma 10.7(iii)) this morphism is surjective. Direct
computation shows that ? ((.4pz,J) = ? (Apl(L)) = h. Apply the Lifting
Lemma 12.4 to lift (?, ?) to a morphism
n:(AV,d)-*(A,d)®(APLJ.
Define ? : (Apl).2 —> A(t,dt) by to >-* I — t, ti *-* 0, ?2 ?-> t; i.e., ? is the face
operator corresponding to (eo,e2) : ?[1] —> ?[2]. Then (??®?)? is a homotopy
from ?? to(/J. D
Notation The set of homotopy classes of morphisms (AV, d) —> (A, d) will
be denoted by [(AV, d), (A, d)\. The homotopy class of a morphism ? will be
denoted by [?]. D
Example 1 Null homotopic morphisms into (A, 0) are constant.
Let (AV, d) be a minimal Sullivan algebra and let (.4, 0) be any commutative
cochain algebra with zero differential. The constant morphism ? : (AV, d) —>
(?,?) is defined by e(V) = 0. We observe that for any morphism ? : (AV,d) —>
U,0),
? ~ ? <^=4> ? = ? .
In fact, suppose ? : (AV, d) —> A <g> A(i, dt) is a homotopy from ? to ? and
write V = (J ^(*) with V(-l) = 0 and d : V(k) —> A^V(k - 1). Assume
k>-\
by induction that ? : V(k - 1) —> A ® ?? ® di. Since di ? di = 0 it follows that
? (A>2V(A; - 1)) = 0.
Choose ? G V(k). Then ?(??) = ?((??) = 0. Hence ?? e (.4®1)?(?®??®???).
Since 0 = ?? = (id ®??)?? it follows that ?? e A <g> ?? ® di. In other words,
Im ? C A® At® dt and ^ = (^(8>??)? = ?. ?
152 12 Sullivan models
Definition The linear part of a morphism ? : {AV,d) —> {AW,d) between
Sullivan algebras is the linear map
?)? : V —> W
defined by: ?? - Qipv 6 A--W, ? e V.
Note that Q(<p) commutes with the linear parts of the differentials: Q{ip)d0 =
Proposition 12.8
(i) If ?? ~ ?? : (AV, d) —> (.4, d) are homotopic morphisms from an arbi-
trary Sullivan algebra then ?{?0) = ? {??).
(ii) If ?? ~ ?? : (AV, d) —> {AW, d) are homotopic morphisms between
minimal Sullivan algebras, and if H1{AV.d) = 0, then Qip0 = ???-
proof: (i) Any element in A{t,dt) can be uniquely written as u = ?0 + ??? +
X2dt + ? + dy, where ? and y are in the ideal / C A{t) generated by <A - t) and
Xi e 1·. If ? is a homotopy from ?0 to ??, define a linear map h : AV —> A of
degree —1 by
?(?) = ??{?) + {??{?) - ??{?)) t - {-l)de*zh{z)dt + ?,
where ? G A ® (/ ? d{I)). A simple calculation gives ?? - ?0 = dh + hd.
(ii) We first observe that V'1 = 0. Write V = \JV{k), with d :
k
V{k) —> A^2V{k - 1). If V^Jfe - 1) = 0 then this implies that d = 0 in Vl{k).
But lmd C A-2V, and so no element of V1(A;) can be a coboundary (except
zero). Since H1{AV,d) = 0 this implies F1^") = 0. The assertion V'1 = 0 follows
by induction.
Now let ? : {AV,d) —> {AW,d)®A{t,dt) be a homotopy from ?0 to ??. Since
V = V^2, it follows that ? : V —> A+W ® A{t,dt). Hence ? : A^*V —>
A-k\V <g> A{t,dt). In particular, it induces a linear map
? : ?+?'/?^? —> (A+W/A^W) ® A{t,dt).
By construction, ? is a map of cochain complexes. However, because {AV, d)
and (AH7,d) are minimal, the differential vanishes in the quotients A+/A-2.
Since A+V = V ? A^2V and A+W = W © A^2W we may therefore identify ?
as a linear map of cochain complexes
The space of cocycles in_{W, 0)®?(?, dt) has the form (IF <g> k · \)©{W <8> A(i)di)·
It follows that (id ·??)? = {id ·??)?; i.e., Q(v?0) = <3(vi). D
(c) Quasi-isomorphisms, Sullivan representatives, uniqueness of min-
_ j„i., oriri fnrtnai spaces.
Sullivan Models 153
Suppose ? : (AW,d) —> (AV,d) is a morphism between Sullivan algebras. If
? is a homotopy between ??,?\ : (??',??) —> (A,d) then ?? : ??? ~ ???. Thus
we can define
?* : [(AV, d), (A, d)} —> [(AW, d), (A, d)] by ?* [?] = [??].
Similarly, if ? : (A, d) —> (C, d) is a morphism of commutative cochain alge-
bras then (? ® ??)? : ??0 ~ ???. Thus we can define
?# : [(AV, d),(A,d)}-+ [(AV., d),(C,d)\ by ??#[?] = [??].
Now suppose given commutath'e cochain algebra morphisms
(A,d)
•l<
(AV, d) > (C, d)
?
in which ? is a (not necessarily surjective) quasi-isomorphism and (AV, d) is a
Sullivan algebra. The Lifting lemma extends to the fundamental
Proposition 12.9 There is a unique homotopy class of morphisms ? : (AV. d) -
(A, d) such that ?? ~ ?. Thus
is a bijection.
proof: We pro\re the proposition first under the additional hypothesis that ? is
surjective. In this case the Lifting lemma 12.4 asserts that ? lifts to a morphism
? : (AV,d) —> (A,d) such that ?? = ?. Thus ry# is certainly surjecth'e.
To show ?# is injecthre, suppress the differentials from the notation (for sim-
plicity). Use the morphisms C ® A(t,dt) Udc'?°' ldc'^\ CxC ^JL ? ? ? to
construct a fibre product, and observe (routine verification) that
id, id ? ·?0, idA -??) : A ® A(t,dt) —> [C <8> A(t,dt)] xCxC (A x .4)
is a surjecth'e quasi-isomorphism of cochain algebras.
Now suppose 70,71 : AV —> A are cochain algebra morphisms, and that ? is
a homotopy from 7770 to 7771. Lift the morphism
(?,7?,7?) : ?^ —> [C® Mt,dt)] xcxC (A x A)
through the surjecth'e quasi-isomorphism (? <g> id, idA -?o, idA -<=i)- This defines
a morphism ? : AV —> A <g> A(t,dt), and it is immediate from the construction
that ? :7? ^7l.
154 12 Sullivan models
Finally, consider the general case, where ? may not be surjective. Let (E(C),6)
be the acyclic cochain algebra of Lemma 12.5. Since E(C) = A(C © 5C) and
since ? : C -^ OC, a surjecth'e morphism of graded algebras, g : E(C) -> C, is
defined by c h-> c, 6c h-> dc. By inspection this is a morphism of cochain algebras.
Consider the morphisms
(A,d) ^^ (A,d) ® (E(C),6) ^> (C,d).
?
Since H (E(C)) = Jk. id -? and ? ¦ g are surjective quasi-isomorphisms. Thus, by
the argument abo\'e, (id -?)# and (? ¦ ?)? are isomorphisms. Since (id -?)? = id,
?# is the bijection immerse to (id ·?)#. Hence ?# = (??·?)#??# is also abijection.
?
Suppose
a:(A,d) —>(A',d)
is an arbitrary morphism of commutative cochain algebras that satisfy H°(—) =
k. Let m : (AV,d) A (A,d) and m' : (AV',d) —> (,4',?? be Sullivan models.
By Proposition 12.9 there is a unique homotopy class of morphisms
? : (AV, d) —> (AV, d)
such that ??'? ~ am.
Definition A morphism ? : (AV,d) —> (AV',d) such that ??'? ~ am is called
a Sullivan representative for a. If / : X -> Y is a continuous map then a Sullivan
representative of Apl(J) is called a Sullivan representative of f
It is follows at once from Proposition 12.6 that Sullivan representatives of
homotopic maps are homotopic morphisms.
As a second application of Proposition 12.9 we deduce the uniqueness of min-
imal models in the simply connected case.
Proposition 12.10
(i) A quasi-isomorphism between minimal Sullivan algebras is an isomorphism
if both cohomology algebras vanish in degree one.
(ii) If(A,d) is a commutative cochain algebra and H°(A) = Je, H1 (A) = 0,
then the
minimal models of (A, d) are all isomorphic.
proof: (i) Let ? : (AV, d) -^ (AW, d) be a quasi-isomorphism between minimal
Sullivan algebras, and suppose H1(AV,d) = 0 = H1(AW,d) Proposition 12 9
then yields a morphism ? : (AW.d) —> (AV,d) such that ?? ~ id. Thus
''·""'' -'1'' nr"i (aeain by Proposition 12.9) it follows that ?? ~ id.
Sullivan Models 155
Now apply Proposition 12.8(ii) to conclude that Q(ip) and Q{il>) are inverse
isomorphisms of V" with W. Since Q(ip) is surjective we ha\-e Wk C ???? +
AH7-'. It follows by induction that Wk and hence ??·'"-?" are contained in
Imtp. Thus ? is surjective.
Use the Lifting lemma 12.4 to choose ? so that ?? = id. Then ? is injective.
But also ??? = ?, so that ?? ~ id. Now the argument just given shows that
? is surjective as well. Thus ? is an isomorphism and, since ?? = id, ? is the
inverse isomorphism.
(ii) Suppose (AV,d) -^ (A,d) ^— (AV',d) are minimal Sullivan mod-
els. Proposition 12.9 provides a morphism ? : (AV, d) —> (AV',d) such that
??'? ~ m. Thus ?(??')?(?) = H(m) — Proposition 12.8(i) — and so ? is a
quasi-isomorphism. Now part (i) asserts ? is an isomorphism. D
Remark In §14, Proposition 12.9 will be extended to the non-simply-connected
case.
Example 1 Wedges.
Since ApL(pt) = Jk (§10(d)) the inclusion of a point j : ? —> X induces an
augmentation
:Apl(X) —>*.
Let (??,??) be based CW complexes, so that ? : Apl(Xo) -> ^ are augmented
cochain algebras. Denote by jQ '· (Xa,xa) —> (\ZQXa,x) the different inclusions
into the wedge. Thus ? ?-> {Apl(Jq)^} defines a morphism
ApL{\/aXa) >[[[) (APL(Xa))
\ a / ft
to the fibre product over Jk of the ApL(Xa). This morphism, which is obviously
surjective, induces the analogue
H'{\JaXa;K) »· ? ? (H*(Xa,Jk)),
\ a / ik
in cohomology and a cellular chains argument (§4(e)) shows this is an isomor-
phism.
Thus the first morphism is a quasi-isomorphism: the fibre product of augmented
commutative models for the XQ is an augmented commutative model for V Xa.
Now suppose ma : (AVa,d) A APL{Xa) are Sullivan models. Then the
quasi-isomorphism
?
15t>
identifies ( []) (AVa,d) as a commutative model for the wedge, \JQXa· 1°
particular, a Sullivan model for ( ? ) (AVQ, d) is a Sullh'an model for the wedge.
Ik
Recall (§10) that two commutative cochain algebras (.4, d) and (A'.d) are
weakly equivalent if they are connected by a chain of quasi-isomorphisms. It
follows from Proposition 12.9 that a Sullivan model m : (AV, d) -^ (^4., d) lifts
through such a chain to yield a Sullivan model m' : (AV.d) -^ (-4',d). /fence
(.4. d) and (A', d) are weakly equivalent if and only if they have the same Sullivan
models. (A common Sullivan model provides an obvious weak equivalence.)
A particularly important case of weak equivalence is identified in the
Definition A commutative cochain algebra (.4, d) satisfying H°(A) = k is for-
mal if it is weakly equivalent to the cochain algebra (H(A), 0). A path connected
topological space, ?', is formal if Apl(X; Q) is a formal cochain algebra.
Thus (A d) and -Y are formal if and only if their minimal Sullivan models can
be computed directly from their cohomology algebras; i.e, if these models are a
'formal consequence' of the cohomology.
The quasi-isomorphisms at the end of Example 1, part (a) of this section,
exhibit the spheres as formal spaces. Example 2 of §12(a) shows that the product
of formal spaces is formal if one of them has rational homology of finite type,
and the example above shows that the wedge of formal spaces is formal.
Suppose now that X has rational homology of finite type. Then the equality
{ApL,k)n = {ApL&)n® Jk defines an inclusion Apl(X;Q) ®qk —> Ap?,(X;k)
of cochain algebras. Since the hypothesis implies that H*(X;Q) ®q Jk -=>
H*{X;3c), this is a quasi-isomorphism. Thus if (AV,d) is a rational Sullivan
model for X then (AV, d) ®q Jk is a Sullivan model for X over k.
In particular, if X is formal then Api(X; k) is formal. In fact the converse is
true:
Theorem [144] [86] If APL(X;k) is formal for some extension field k D Q
then X is a formal space.
We shall not reproduce the somewhat technical proof, and we shall not often
need to apply the theorem except in the case of geometric examples arising from
C°° differential forms.
(d) Computational examples.
By using Sullivan algebras and computing homotopy classes of morphisms it
is possible to illustrate interesting phenomena and, sometimes, to completely list
all homotopy classes. Since Sullivan algebras (of finite type and with H1 = 0)
Sullivan Models 157
are always the models of simply connected spaces (§15), such phenomena can
always be realized geometrically.
We shall present Sullivan algebras in the concise form (AV, d) = ?(??, v2.... ;
dv\ =,...) and we shall only indicate the degree of a generator Vi when it is not
implicit in the formula for dv{.
Example 1 (AV,d) = A(x,y,z;dx = dy = 0, dz = xy), deg a; = 3, degy = 5.
A basis for the cohomology H — H(AV,d) is provided by 1, [a:], [y], [xz], [yz]
and [xyz], and the only non-trivial products are given by
[x] · [yz] = [xyz] = ~[y}[xz\.
Consider all the possible morphisms ? : {AV, d) —>¦ (H, 0). For degree reasons,
we must have
?? — ? [?], tpy = ?[y], and ?? = 0, some ?, ? € Jk.
These morphisms induce distinct maps in cohomology, and hence represent dis-
tinct homotopy classes. Thus this provides a complete list of the elements in
[(AV,d), (H,0)]. Note that none of these morphisms is a quasi-isomorphism so
that (as with Example 7 in (a)), (AV, d) and (H, 0) are not weakly equivalent:
(AV,d) is not formal. ?
Example 2 Automorphisms of the model of S3 x S3 x S5 x S6.
Note that the tensor product of commutative models (Sullivan or otherwise)
is a commutative model of the topological product, as follows from §12(a). Thus
S3 ? S3 ? S° ? S6 is formal. In Example 1 of part (a) in this section we computed
the minimal Sullivan models of spheres. This shows that the minimal model of
S3 x S3 x S5 ? Se has the form (AV,d) = A(x,y,z,a,u;dx = dy = dz = da =
0, du = ?2), with degar = degy = 3, degz = 5, dega = 6.
Define an automorphism, ?, of this model by:
?? = ?, i/py = y, ?? = ?. ?? = ?, ??? = u + xyz.
Then ?(?) = id and Q(<p) = id but ? is not homotopic to the identity.
Indeed, suppose ?? ~ ?? : (AV, d) —)¦ (AV, d) via a homotopy, ?. For degree
reasons, ?? = a <g> fx (t) + xy <g> /2(i) + ? ® fs(t)dt. Similarly, ?? = u <g> gx (t) +
xyz ® g2(t) + za® gz(t). From ??? = 0 deduce /i, f? € Jk. If ?$? = a it follows
that fx = 1 and /2 = 0. In this case the equation d<&u = (??J implies that
Pi = 1, and dg-2 = 0. Thus go € k and ??? — ???? = ???. In particular, ? is not
homotopic to the identity. D
Example 3 (AV, d) = A(a, x, b; da = dx - 0, db = ax), deg ? = 2, deg ? = 3.
Let / be the ideal generated by a2, xb2, ab2 and 63. It is preserved by d, so
that a commutative cochain algebra (A, d) is defined by .4 = AV/I.
A basis for ? (A) is given by 1, [a], [a:], [ab] and [xb]. In particular, H+(A) ¦
H+{A) = 0, and so ? (A) ^ H*(S2 VS3VS6V S7;Jk). However (A,d) and
#*(S2 V 53 V S6 V S7; it) are not weakly equivalent.
158 12 Sullivan models
Indeed if they were, a minimal model for (A, d) would also be a minimal model
for H(A, d). The construction process of Proposition 12.2 produces a minimal
model (AW, d) -=> (A, d) that is given in degrees < 5 by ?(?, u, x, b; da = 0, du =
a2,dx = 0, db = ax). But any morphism from (AW,d) —> (H(A,d),0) sends
6 t-4 0 for degree reasons, and hence kills the cohomology class [ab]. ?
Example 4 A(x,y,z,u;dx = dy = dz = 0,du = xm + ym — zm), degar =
degy = degz = 2, m > 3, h = Q.
A morphism (A(a;,y, z, «),d) —>· (??, 0), dega = 2, is given by ? ? ??,
y M· ??, ? ?· ??, where Am + ?™ = ?7" and ?, ?, ? G Q. By Fermat's last
theorem, one of ?, ?, ? is zero. This illustrates how quickly number theoretic
questions intervene in these computations. ?
Example 5 [66] Two morphisms that homotopy commute, but are not homo-
topic to commuting morphisms.
Define a minimal Sullivan algebra (AV,d) by specifying the differential in a
basis of V as follows:
V3 V5 Ve V7 Vn
??,?2,?? y ? ? u>i,u>2
dxi = 0 dy = ?2?3 dz = 0 dv = yx-i dw\ = z2, dw2
Define two automorphisms ?, ? : (AV, d) —» (AV, d) by requiring
?? = ? + ?\?2, ???? = w\ + 2tu2, ? = identity on the other generators,
and
¦??? = x\ + xz, i\)Wi = ui2 — zy, ? = identity on the other generators.
Then a homotopy ? : (AV, d) —> (AV, d) <g> A(i, dt) from ?? to ?? is given by
= ?? + x3, ?? = ? + ???2 + X3X2 + ydt,
= ?? 4- 2tu2 - lyzt 4- 2xiudi 4- 2x3vtdt,
?\ + xz)vdt,
and ? = identity on the other generators. (This is a longish, but easy computa-
tion.)
On the other hand, ???~?' then ? (?1) = ? (?). This implies that ?'?? = ?,·.
Hence 0 = d(<p—<p')y. There are no cocycles in degree 5, so ?'y = ipy = y. Again,
because ? (?1) = ? (?) we have ?'? = ?? + ??2?3, some ? € Je. Similarly, if
?' ~ ? then ?'Xi = ???, ?'y = ?? and ?'? = ?? + ??2?3, some ? € Jk. A
computation now shows that ?'?'? ? ?'?'?.
The reader is challenged to show that H(AV, d) is a finite dimensional algebra,
with top cohomology class of degree 49.
Example 6 [57] Sullivan algebras homogeneous with respect to word length.
Sullivan Models 159
The differential in a Sullivan algebra (AV, d) is said to be homogeneous of
degree k with respect to word length if d : V —> Ak+1V. Thus if we let
ffp,Q c Hv+q{AV) be the subspace represented by cocycles in APV then Hn =
0 H™ and H™ · Hr's C Hp+r^+s.
p+q=n
On the other hand, suppose a graded vector space V is presented as a di-
oo oo
rect sum of graded vector spaces: V = ? Vm. Then AV = 0 (AV)m,
m=0 m=0
where ???···??? € (AV)m if Vi € Vm. and Era, = m. Thus if (AV,d)
is a Sullivan algebra and d : Vm —» (AF)m_i, m > 0, then a 'lower grad-
oo
ing' Hr(AV, d) = 0 H^AV, d) is induced in the cohomology algebra. Again,
771=0
Now fix k > 1 and any graded vector space ? of the form ? = {??}{>2· We
shall construct a minimal Sullivan algebra (AV, d) homogeneous with respect to
word length of degree k and with the following properties:
• V is a grad?d vector space and V = {Vl}i>2-
oo
• V = 0 Vm and d : Vm -> {Ak+1V)m_r
771=0
• Vo = ? and the inclusion induces an isomorphism ? -^ H1'*(AV).
= 0.
For this, set Vo = Z, and d = 0 in Z. Next, construct <i and Vm induc-
tively so that d : Vm+i A {Ak+1V<m)m nkerd. Then H(d) : Vm+i -=>
i?4+1>* (AV<m,d). Now the first three properties above are immediate, as is
the fact that Hk+1>*(AV,d) = 0.
It remains to see that H>k+1'*(AV, d) = 0. This is proved inductively as
follows. Suppose v\,..., vr € V satisfy dvi = 0 and dv{ € A(v\,..., Vi_i). Then
we can divide by vi,... ,vr to obtain a quotient Sullivan algebra (AW, d): here
we may identify W with any complement of Jkv\ ? · · · 0 lkvT in V. We call
(AW, d) an r-quotient, and show that:
• For any r-quotient (AW,d), H^k+l>*(AW,d) = 0. A2.11)
In fact suppose A2.11) is false. Then there is a least degree ? in which it fails
and a_least r = ro for which it fails in that degree. Thus for any s-quotient
(KZ, d) and for any ? > k + 1 we have:
Hp'Q(AZ,d) = 0 iip + q <n or if p + q = n and s < ?0. A2.12)
Now suppose (AW, d) is any r0-quotient and ? is a cocycle of degree ? in APW
for some ? > k + 1. We shall show ? is a coboundary, thereby contradicting the
hypothesis that A2.11) fails.
160 12 Sullivan models
Suppose first rc, > 1. Then there is an (r0 — 1) quotient of the form (Av®AW, ?)
projecting to (AW,d). In particular, ?A <g> ?) = vu, some ? € Av <g> ? VF. Now
i/ degv ? odd then 5A ? ?) = ? <g> ? with ? G ??-F. Moreover ??? = 0,
deg? < 7? and ? € ?-?+1 W. Our hypothesis above implies that ? = ??? and so
?A ® ? + ? ® ?) = 0. On the other hand, ?/ degv is even then ?<5? = 0. Thus
? is a cocycle of degree < ? and word-length > k + 1 in an (r0 - l)-quotient.
Hence ? = oSP and EA ® ? - ??) = 0. In either case ? lifts to a cocycle of
degree il and word-length ? in an (ro - l)-quotient. Our hypothesis implies that
this cocycle is a coboundary; hence ? is a coboundary in {AW, d).
Finally, suppose r0 = 0. In this case (AW,d) = (AV,d) and J2*+1'*(AV) = 0
by construction. Thus we may suppose ? > k + 2. Now for s > 1 any s-quotient
(AZ, d) is the projection of an (s — l)-quotient of the form (Av <S> ??,?). We
shall show that if the image of ? in (AZ, d) is a coboundary then so is its image
in (Av <S> ??, ?). Since (clearly) ? maps to zero in some g-quotient it will follow
that ? itself is a coboundary.
Let ? be the image of ? in Av <g> AZ. Its image in AZ has the form d~T and so
? — ?A <g> ?) = vCt. If ? has odd degree we may write ? - ?A ® ?) = ? ® ?; here
<?? = 0 and ? is a cocycle of word-length ? — 1>& + Iinan s-quotient. Since
deg? < ? we have ? = du' and ? = ?A <g> ? — ? <g> ?'), //? /ias euen degree
then ?? = 0 and ? is a cocycle in an (s — l)-quotient of word-length > k + 1
and degree < n. Thus ? = ??.' and ? = ?A <g> ? + ??'). ?
Example 7 Sullivan models for cochain algebras (H, 0) wii/i trivial multiplica-
tion.
Let H = h® H-2 be a graded algebra with trivial multiplication: H+ · ii+ =
0. Regard if as a cochain algebra with zero differential.
In Example 6 we constructed word-length homogeneous Sullivan algebras.
Here we consider the case k = 1 and ? = H+. Then the construction of Exam-
ple 6 gives a Sullivan model of the form (AV, d) with
V = H+®V?®¦¦¦®Vm®¦¦¦
d : Vm -> (A2F)m_i ,
and
H^iAV, d) = H+ and H^2>*(AV, d) = 0 .
It follows that dh'iding by A-2V and by V>\ defines a quasi-isomorphism
(AV, d) ^ (H, 0)
which exhibits (AV,d) as the minimal Sullivan model for (H, 0). ?
(e) Differential forms and geometric examples.
In this topic we take Jk = R.
Let M be a smooth manifold. In §11 we showed that Adr(M) was weakly
eauivalent to Apl(M;R). Thus the Sullivan models of M are identified with the
Sullivan Models 161
Sullivan models of Adr(M). This represents a significant strengthening of de
Rham's theorem, which asserts that H*(M;R) = H (ADr(M)).
In particular suppose M is simply connected and has finite dimensional ratio-
nal homology, and let (AV.d) be a minimal Sullivan model for Adji(M). Since
this is also a model for Apl(M; M) we may conclude (taking for granted results
announced in §12 and §13 and to be pro\red in §14 and §15) that
• H(AV,d) = H*(AI;R) as graded algebras.
• Whitehead products may be computed from the quadratic part of the
differential, d.
Finally, with these hypotheses Adr(AI) is connected to Apl(M;Q)®R by quasi-
isomorphisms of commutative cochain algebras (§11). It follows that (AV, d) =
(AW, d) <g> K, where (AW, d) is a rational minimal Sullivan model for M.
Since geometric restrictions on M (e.g. M admits an Einstein metric) are
often expressed in terms of differential forms, it is natural to expect that these
could lead to information about the model and hence to information about the
homotopy groups of the manifold. Unfortunately results of this nature have, so
far, proved to be elusive.
Now suppose a Lie group G acts on AI, and let Aor(M)g denote the sub
cochain algebra of differential forms invariant under translation by ? € G. A
theorem of E. Cartan [32], [70], asserts that if G is compact and connected, then
the inclusion Adr(AI)g —> Adr(AI) is a quasi-isomorphism, and so Adr(M)g
is also a commutative model for AI.
Example 1 The cochain algebra Adr(G)g of right invariant forms on G, and
its minimal model.
Let g be the Lie algebra of the group G, identified with the Lie algebra of
right invariant vector fields on G. Regard g" = Hom(g; K) as a graded vector
space concentrated in degree 1. Let G act on itself by right translation. Then
Adr(G)g — (Ag'.d), and the formula for the exterior derivative gives
(??;??) = (?[??)) ^
Because g*1 generates Ag" and because d is purely quadratic this formula deter-
mines d.
Although AgE is a free commutative graded algebra, the cochain algebra (Ag3, d)
does not, in general, satisfy the nilpotence condition required for Sullivan alge-
bras. In fact, it is easy to see that (Agc, d) is a Sullivan algebra if and only if g
is a nilpotent Lie algebra.
On the other hand. G is always homeomorphic to the product of a compact
Lie group with some W1 [32]. In particular (as with any compact manifold,
143], [69]) Ht(G;R) is a finite dimensional vector space. Thus the theorem of
162 12 Sullivan models
Hopf (Example 3, §12(a)) shows that H*(G;R) is an exterior algebra on a finite
dimensional vector space ? concentrated in odd degrees. This gives a minimal
Sullivan model
(??,?) ^ ADR(G),
which contrasts with the morphism (Ags, d) —> Adr(G). ?
Example 2 Nilmanifolds.
Let G be a nilpotent connected Lie group and let ? be a discrete sub-group of
G such that the quotient space X — G/T is compact. The covering projection
? : G —> X induces an isomorphism AdrX — (ApRG)r (= the complex of
right ?-invariant differential forms on X). Thus we may regard {AdrG)g as a
subcochain algebra of AqRX. In [130], K. Nomizu proved that this inclusion is
a quasi-isomorphism.
In this case the Lie algebra g of G is nilpotent and so (Ag5,c() is a minimal
model of X over the real numbers. Obviously, d = 0 if and only if the Lie
algebra g is abelian. In this case X = 51 ? · ¦ · ? S1. In particular, X is formal.
The converse is true. Indeed, assume that there exists a quasi-isomorphism
(?(??,?·2,... ,Xk),d) -^> H*(X;M). Then ? is obviously surjective, and since
the product X\X2 · · · xk is a cocycle which cannot be a coboundary, ? is injective.
This in turn implies that d = 0.
This result has been proved in many different manners: [23], [39], [50], [53],
[88], [114]. ?
Example 3 Symmetric spaces are formal.
Suppose ? is an involution of a compact connected Lie group G, and that ? is
the connected component of the identity in the subgroup of elements fixed by ?.
Then G/K is called a symmetric space of compact type. By Cartan's theorem,
ADR(G/K)G -^ADR(G/K).
An argument of E. Cartan shows that the differential in Adr{G/K)g is zero,
thereby exhibiting G/K as a formal space. It is interesting to note that this is
still the only proof available of the formality of G/K.
Cartan's argument runs as follows: observe that r induces an involution ? of
G/K and that ADR(a) restricts to an involution of Adh{G/K)g. Since the ac-
tion of G on G/K is transitive. Adr{G/K)G may be identified with asubalgebra
of AT?{G/K) = A(g/E)\ Let ?' :TG—>TG and ?' : TG/? —> TG/K denote
the derivatives of r and ?. Since ? is an involution and t = {h G g | ?'h = 1}, it
follows that ?' = - id in g/L Hence ADR{o) = {-\)Hd in APDR(G/K)G. Since
Adr{o) commutes with d, d = 0. D
Example 4 (Deligne, Griffiths, Morgan and Sullivan [42]) Compact Kahler
manifolds are foimal.
Suppose M is a complex manifold with operator J : TX(M) —> TX(M)
satisfying J2 = — 1, ? e M. Let ( , ) be a Riemannian metric such that
(JC, J77) = (?,?). Define ? G A2DR(M) by ?(?,?) = (JC,??). ?/ is called a
Kahler manifold if ( , ) can be chosen so dw = 0.
Sullivan Models 163
Given a Kahler manifold, put dc = J~ldJ : ADR{M) —> ADR(M). Then
((fcJ = 0 and dcd = ddc. In [42] the authors show that d : keidc —> Imf and
that the obvious inclusion and surjection
Adh(M) A (kerdc,d) -A (ker cf/ Im dc, 0)
are quasi-isomorphisms. This exhibits M as formal.
Here, again, the only known proof of formality for Kahler manifolds proceeds
via analysis using Adr[M). ?
Example 5 Symplectic manifolds need not be formal.
A symplectic manifold is a 2n-manifold M equipped with ? € A?DR(M) sat-
isfying ?? = 0 and ?" ^ 0, ? € ?·/. Thus Kahler manifolds are symplectic.
Now let X = Nz\ ? be the manifold constructed out of the 3x3 upper
triangular matrices as described in Example 2. The Lie algebra of ? has the basis
h\,h.2,h.2, with hz central and [??,/12] = ^3- Thus the minimal Sullivan model
constructed in Example 2 has the form (A(x, y, z),d) with dx = dy = 0, dz — ? Ay
and x,y,z of degree 1. Note that this is not formal (as is already observed in
[107]; p261) since the algebra H*{X; E) is not generated by ?1 (?; ?). (See also
Benson and Gordon [23], Cordero, Fernandez and Gray [39], Felix [50], Felix
and Halperin [53], Lupton and Oprea [114], Hasegawa [88])
Put ? = ? ? ?. Then the inclusion
(A(x,y,z),d) ® (A(x',y',z'),d) A ADR{M)
is a minimal Sullivan model for M. Hence, as above, M is not formal. Let ? be
the image in A2DR{M) of the cocycle ? Ax' + yAz + y' Az'. Since ?3 ? 0 and ?3
is left ? ? iV-invariant it follows that ?3 is not zero at any point of M. Hence
(?,?) is a non-formal symplectic manifold. ' ?
Recently I.K. Babenko and I.A. Taimanov, [17], have constructed for any
? > 5 infinitely many pairwise non homotopy equivalent non formal simply
connected symplectic manifolds of dimension 2n. In fact, as proved, by S.A.
Merkulov, [124], a symplectic 2n-manifold M is formal if the de Rham co-
homology of M satisfies the hard Lefschetz condition: i.e. the cup product
[??] : Hn~k(M; 1) —> Hn+k(M; R) is an isomorphism for any k < n.
Exercises
1· Let (AV, d) be a Sullivan model of S2 V S3. Prove that there exists a quasi-
isomorphism ? : (AV,d) -> H*(S2 V S3;Q). (Cf. d)-example 7). Determine the
restriction of d to Vn for ? < 6.
2. Prove that if X and Y are formal spaces so are X V ?, ? ? ?, ? ? ? and
^ * Y. Determine the minimal Sullivan models of Ss ? CP6, S8 A CP6 and of
S8*CP6.
164 12 Sullivan models
3. Let (A,(Ia) be any commutative graded algebra and (f\Z,d) -> H(A,Aa) a
bigraded model as defined in f )-example 7. Prove that there exist a differential
D and a quasi-isomorphism ? : (AZ,D) ~> (Ad.4.) such that D = Dx + Do +
... + Dt + ... with DiZ1; C (/\Z)^+l and Dx = d. {see [86].)
4. Construct a surjective quasi-isomorphism
with duo; = 0 = du^ji dxi = v-> + wo. dxz = v4 + W4 + vow?-. dx5 = v^wo +
dx7 = u4uL, dy2 = 0, dy4 = 0, Jz5 = 2/2By4 - y|) and dz7 = y4(y^ - y4).
5. Let (A,d) be a commutative differential algebra and for ? > 1 denote by
A(n) the subalgebra defined by
? Ak nk<n
A(n)h = < dAn if k = ? + 1 .
[ 0 if jfe > ? + 2
Pro\re that if (,4, d) is formal so is (A(n).d). Let (AV,d) be a Sullivan model of
a 1-connected CW complex. Construct a commutative model of the n-skeleton
of X. (Cf. a)-example 6).
6. Let (A, d) be a commutative differential graded algebra and o2· € Ani, i =
0,1,2, be cocyclessuch that dai2 = ???2 ,da2s = a2a3 . Prove that (-l)degaia1a23
- a\2a3 is a cocycle; its cohomology class (which depends on the choice of ??2
and ?23) is called a Massey product. Construct a non zero Massey product
in the cohomology of the minimal model (? {ae,a\o,bi\,bi5,b\o) ,d) such that
dbn = ag , dbi$ = a6 a10 , dbi9 = aj0. Using the bigraded model of d)-example 7
prove that a formal space does not admit non trivial Massey products.
7. Let (AV, d) be a Sullivan minimal model and ? ,? : (aV,d) —> (A,d) two
morphisms of commutative differential graded algebras. Assume that for any
? > 1, the restictions of ? and ? to (AV-n.d) are homotopic Pro\^e that ? and
?/> are homotopic. There are no phantom maps in rational homotopy theory!
8. Let M and ? be compact connected oriented ? -dimensional manifolds. Prove
that if M and TV are formal so is the connected sum M#N (see §ll-exercise 3).
Compute the mimimal model of (S3 ? 53)#CF3 up to dimension 12.
13 Adjunction spaces, honiotopy groups and
Whitehead products
In this section, the ground ring is a field 3c of characteristic zero.
In applying Sullivan models it is important to be able to compute directly
the models of geometric constructions from models for the spaces used in the
construction. For example, in §12 we saw that the tensor product of models
was a model of the topological product, while in §14 we will see how to model
fibrations.
A topological pair (Z, F) and a continuous map /:!*—> ?" determine the
topological pair (?" U/ Z, X) in which A' U/ ? is the adjunction space obtained
by attaching ? to X along /. These define the commutative square
Y
X
?
Sz
X U/ ?
A3.1)
Analogously, if
(Cd) A
are morphisms of commutative cochain algebras then the fibre product (C ? ? .4, d)
fits in the commutative fibre product square
(B;d)
(C,d)
(A,d)
A3.2)
(C xs ,4, d),
and ?? is surjective if ? is. Note that in C.1) we have Z/Y = X U/ Z/X
while in C.2) we have keri/> = ker^c- If (Z,Y) is a relative CW complex
then the first equality implies (by the Cellular chain models theorem 4.18) that
H, (Z, Y\ Jk) A H* (X of Z, X; k).
Our first objective in this section is to show that
• Suppose ? and ? are Sullivan representatives for f and for i and one of
?, ? is surjective. IfH.(Z,Y;k) A H»{X U/ Z;k) then [C xB A,d) is
a commutative model for X U/ Z.
Thus a Sullivan model for the adjunction space may be computed directly from
the Sullivan representatives ? and ? of / and i, provided that ? is surjective.
We shall then construct particular commutative models for the geometric con-
structions: cone attachments, cell attachments and suspensions.
166
13 Adjunction spaces, homotopy groups
Next, suppose (AV,d) is a minimal Sullivan model for a simply connected
topological space X. Our second objective in this section is to construct a
natural linear map
vx : V —> Horn (vr.(*),*)
and then to use the model of a cell attachment to show that:
• ?? transforms the quadratic part of the differential in AV to the dual of
the Whitehead product in ?*(?).
Later (§15) we shall show that under mild hypotheses ?/? is an isomorphism, so
that this transformation is in fact an identification.
This section is organized into the following topics:
(a) Morphisms and quasi-isomorphisms.
(b) Adjunction spaces.
(c) Homotopy groups.
(d) Cell attachments.
(e) Whitehead products and the quadratic part of the differential.
(a) Morphisms and quasi-isomorphisms.
Morphisms (C,d) -A (B,d)
into the fibre product square
- (A,d) of commutative cochain algebras fit
(B,d) -. (A,d)
V :
and a commutative diagram
(C,d) 1
(C,d)
(C xBA,d) ,
(B,d)
(A,d)
extends by G,0) : (C xB A,d) —>· (C xB' A',d) to a commutative cube con-
necting the corresponding fibre squares. We shall require
Lemma 13.3 If ?-, ?, a are quasi-isomorphisms and if one of ?, ? and one
of ?',?' are surjective then G,0) : (C xB A,d) —> (C xB· A',d) is a quasi
isomorphism.
Sullivan Models
167
proof: If ? and ?' are both surjectu'e then a restricts to a quasi-isomorphism
ker?/> -=» keri//. Now use the row exact diagram
0
0
kerip —~ C ? ? A
C
ker0' - C xB- ?' — C
0
0
to conclude that G, a) is an isomorphism.
The other case to consider is that ?' and ? are surjective. As in §12(b) choose
a surjective commutative cochain algebra morphism ? : (?, ?) —> {B',d) with
H(E) = k, and consider
C -4— B' -^- A'
?'? ?
The argument above shows that G, ??) : (C xb A, d) —> (C ??· (?' ? ?), d)
is a quasi-isomorphism. Since ?' is surjective the same argument shows that
(id, X) : (C Xb' A',d) —> (C Xb1 (A' <g> E),d) is a quasi-isomorphism. Hence
so is G, a). ?
In general a commutative cube
of morphisms of commutative cochain algebras will be regarded as a morphism
?* —>¦ V from the top square to the bottom one. If the vertical arrows are all
quasi-isomorphisms this is called a quasi-isomorphism of squares and written
? -—* V. Two commutative squares connected by a chain · -=*· · «=- · · · · · <^L
• of quasi-isomorphisms are called weakly equivalent (as already defined at the
end of §7). Thus the conclusion of Lemma 13.3 asserts that G,0) defines a
Quasi-isomorphism between the two fibre product squares.
168 13 Adjunction spaces, homotopy groups
Next consider a homotopy commutative diagram
(AV,d) -*- (B,d) -?¦ (AW,d)
(C,d) -p (G,d) -viF.d)
of morphisms of commutative cochain algebras, in which (AV,d) and (AW,d)
are Sullivan algebras.
Lemma 13.4 Suppose ?, ?, y are quasi-isomorphisms and that one of ? and ?
and one of ? and ? are surjective. Then the fibre squares corresponding to ?, ?
and to ?, ? are weakly equivalent.
proof: For definiteness take ? to be surjective. Suppose first that both ? and
? are surjective. Then we construct morphisms a' ~ a and 7' ~ 7 such that
replacing ? by a' and 7 by 7' makes the diagram above commutath-e. Thus in
this case the Lemma follows from Lemma 13.3.
To construct a', let ? : (AW,d) —> (G,d) <8> A(?, dt) be a homotopy from ??
to 77a: ?0$ = ?"? and ?? ? = ??, as described in §12(b). Use ?\ to form the fibre
product G ® ?(?, dt) ? ? ?. Then, in the diagram of cochain algebra morphisms
F <g> ?(?, dt)
(MV, d) ——-*¦ G ? ?(?, dt)xGF ,
(?,?)
the vertical arrow is a surjective quasi-isomorphism. Use the Lifting lemma 12.4
to lift (?,?) to ? : (AW,d) —> F®A(i,df), and set a' = ????· The morphism
7' is constructed in the same way.
Finally suppose ? (for definiteness) is not surjective. Use the surjective trick
(§12(b)) to extend ? to a surjection ?-? : (C,d) <S> (?,?) —> (G,d), with
H(E, ?) = Jk. Then the fibre square for ? ¦ ? and ? is weakly equivalent to the
fibre square for ?, ? by Lemma 13.3 and weakly equivalent to the fibre square for
?, ? by the argument above. D
(b) Adjunction spaces.
Suppose that i : Y —> ? is the inclusion of a topological pair (?, ?), and that
Sullivan Models 169
/ : Y —> X is any continuous map. Then we ha\re the commutative square
Y — ? ApL(Y) - ApL(Z)
f
fz , and the V :
commu-
Ar —- Ar Uf ? tative -4??,(?) :— APL(X Uf Z)
model
The square V determines the morphism
(ApL(ix),APL(fz)) : APL(X Uf Z) -> APL(X) xApLiY} APL(Z)
which (together with the identities) defines a morphism from V to the fibre
product square.
Proposition 13.5 If H,(Z,Y;k) -=> H,(X Uf Z,X;k) then the morphism
(ApL(i.x),ApL(fz)) is a quasi-isomorphism. Thus the fibre product is a com-
mutative model for the adjunction space.
proof: Since H,(Z,Y:k) -=» H,(X U/ Z,X;k) it follows that APL(X Uf
?,?) -^> ???,(?,?). Thus in the row exact diagram
0 ^ Apl (X Uf Z, X) ^ APL (X Uf Z) ^ APL(X) ^ 0
0 >~APL(Z,Y) ^Apl(X) xApl(Y) Apl{Z) * APL(X) ^0
t"he central arrow is a quasi-isomorphism. ?
Proposition 13.5 replaces the geometric adjunction space by the algebraic fibre
product. Using Lemma 13.4 we can now pass to Sullivan models. Thus suppose
?, ? and ? are path connected, that
mx : (AV, d) —> APL(X) , m : (AU, d) —> APL(Y) and mz : (AW, d) —> APL(Z)
are Sullivan models and that
(AV, d) A (AU, d) ? (AW, d)
are Sullivan representatives for / and for i.
Proposition 13.6 If Ht(Z,Y;k) A H,(X U/ Z,X;Jk) and if one of ?,-? is
surjective then V is weakly equivalent to the fibre product square
(AU,d)* (AW,d)
(AV,d) * (AV xau AW,d) .
170 13 Adjunction spaces, homotopy groups
In particular, (AV x,\u AW,d) is a commutative model for X U/ Z.
proof: This is an immediate translation of Lemma 13.4, given Proposition 13.5.
?
Now consider the very important special case of adjunction spaces given by
attaching a cone. Recall that the cone on a topological. space Y is the space
CY = (Y x I)/(Y x {0}). We identify Y as the subspace ? ? {1} C CY. Then,
given any continuous map / : Y —> X we attach the cone CY to X along / to
form X LJ/ CY.
Now, suppose X is path connected. We shall use a different technique to
construct a commutative model for XU/CY. Let ma : (AWa,d) ^> ApL{Ya) be
Sullivan models for the path components Ya of Y, and put (B,d) = Y\(AWa,d).
a
Then l\APL(Ya) = APL(Y) and m = l\ma : (B,d) -=» APL(Y) is a quasi-
a a
isomorphism. Let ?? : (AV, d) —> (AWa,d) be Sullivan representatives for
fa — f \y Wltn respect to a Sullivan model ??? : (AV,d) —> Api(X), and put
y((
Finally, as usual, let ??^? ·' A(t,dt) —> k be the morphisms 11—> 0,1. Thus
kere0 is the ideal A+{t,dt). Use the morphisms
(AV, d) A (B, d) 4^- [k @[B® A+(t, dt)} , d) A3.7)
to construct the fibre product AVxB (Jk®[B ® A+(t,dt)]).
Proposition 13.8 The fibre product square for A3.7) is weakly equivalent to
the commutative adjunction space for XUfCY. In particular the cochain algebra
AV ??(?;?[?® A+(t,dt)]) is a commutative model for X U/ CY.
proof: Use Proposition 13.5 to identify APl(X) Xapl(Y) Apl(CY) as a com-
mutative model for Xo/ CY. Let ix denote the inclusion y ?—> (y, 1) of Y in CY
and in ? ? / and also of {1} in /, and notice that the chain of quasi-isomorphisms
Apl{CY.pt) A APL (? ??,?? {0}) <?- ApL(y) ? ApL (/; {?})
is compatible with the surjections Apl(ii) and id<8>ApL(ii). Regard the iden-
tity of / as a 1-simplex. Restriction to this simplex is a quasi-isomorphism
ApL(I, {0}) —>¦ A+(i,di) which converts id®APL{i\) to ??®??. Since APL(CY)
= k © ApL{CY,pt) we obtain a chain of quasi-isomorphisms connecting
APL(X) Xapl(Y)api<{CY) with Apl(X) xApL(Y) {k ®[APL(Y) ® A+(t,dt)])-
On the other hand, there are homotopics ????? ~ ApL(fa)mx. A choice of
Sullivan Models 171
these defines a commutative diagram
APL(X) - ^ (.W\d)
ApL(Y) -.— ????.??)®?(?,<??)) ?—.. APL(Y) -?- (B.d)
and this connects -4Pi,(X) xApl{y) {k @ [APL(Y) <g> A+(?,d*)]) to
AV xB (J: ? [? <g> ?+(?,d?)]) by a chain of quasi-isomorphisms too. ?
As a special case of Proposition 13.8 we prove
Proposition 13.9 The suspension. ??, of a well-based topological space (Y, yo)
is formal, and satisfies H+{EY;Jk) ¦ ?+(??;&) = 0.
proof: Apply Proposition 13.8 to the case of the constant map / : Y —> {pt} to
obtain a commutative model for {pt}ofCY of the form kx?(k © [? ® A+(t, dt)]).
But this is just the cochain algebra Jk © [B <8> J], where J = kerei ? ?+(?, dt).
Now J is the ideal generated by t(l — t) and dt and thus the inclusion kdt —> J
is a quasi-isomorphism. It follows that Jk®[B <S> dt] includes quasi-isomorphically
in k ? [? <8> J]. Let ? C ? ® dt be any subspace of cocycles mapping isomor-
~phically to H(B<S>dt). Since (B®dt) -{B®dt) C B®(dtAdt) = 0, the inclusion
(k ? ?, 0) —>· Je © [5 <g> J] is a cochain algebra quasi-isomorphism, exhibiting
k© ? as a. commutative model for {pt} U/ CY in which ? ¦ ? = 0.
Finally, {pt} U/ CF = CY/{Y x {1}), which is homotopy equivalent to ??
since (Y,yo) is well-based (§l(d)). ?
(c) Homotopy groups.
Suppose / : (Y, *) —> (X, *) is a continuous map between simply connected
topological spaces. Recall that a choice of minimal Sullivan models,
mx : (AV,d) A APL{X) and my. (???, d) A >1FL(}')
determines a unique homotopy class of morphisms ?} : (XV,d) —> (AW,d) such
that ApL(f)mx = myipf. Moreover, Proposition 12.8(ii) asserts that these
Sullivan representatives of/ all have the same linear part: Q(ff) is independent
of the choice of ?/. This will therefore be denoted by
QU) ¦ V -+ W.
Note that (Proposition 12.6) Q(f) only depends on the homotopy class of /.
We use this construction to define a natural pairing, ( ,· ) between V and
?*{?), depending only on the choice of ??? : (AV,d) —> Apl(X).
First, recall the minimal Sullivan models m^. : (A(e),0) —> ApiJ(Sh), k odd.
and rrik : (A(e,e'),de' = e2) —> ApL(Sh), k even, as constructed in Example 1,
172 13 Adjunction spaces, homotopy groups
§12(a). Because H*(Sk:lk) is so simple, the morphisms mk are determined up to
homotopy by the condition (H*(mk)[e\, [Sk]} = 1, where [Sk] is the fundamental
class defined in §4(c) and ( , ) denotes the pairing between cohomology and
homology (§5).
Now suppose a ? 7?^(?') is represented by ? : (Sk, *) —> (X, *). Then Q(a) :
Vk —> Ik ¦ e depends only on a and the choice of the morphism ??? : (AV, d) —>
Define the pairing
<—,-): F x ??,(?) —>· k A3.10)
by the equations
Q(a)v , ? ? Vk,
(vi d\e ?=
1 0 , deg ? -?- deg a .
It is immediate from the definition that for / : (Y, *) —> (?", *) as above,
(Q(f)v;?) = (?;?.(/)?), ? € V, ? G ??.(?).
Lemma 13.11 The map (—;—} is bilinear.
proof: The linearity in V is immediate from the definition. To show linearity in
?*(?), consider the space SkvSk. Let io,ii be the inclusions of the left and right
spheres and let j : Efc, *) —> (Sk V Sk, *) satisfy [j] = [i0] + [i{\ in irk(Sk V 5*).
The linear maps Q(io), Q(i\) and Q(j) can be computed as follows. Let ???,??? be
the basis for Hk(Sk V Sk;3e) defined by (u;o,^(to)[5fc]) = 1 = (w1,H.(i-l)[Sk])
and (???,??,^?)^]) = 0 = (w1,H,(i0)[Sk}). Then a minimal Sullivan model for
Sk V Sk is given by
where eo,e\ are cocycles of degree k, the remaining generators have greater
degree, and H(m)[e\] = ??, ? = 0,1.
Thus a Sulli\ran representative ?0 for i0 satisfies ?(??)[??] = [e] and ?(??)[??]
= 0. Since the models have no coboundaries in degree k this implies </?oeo = e
and </?oei = 0; i.e. Q(io)eo = e and Q(i'o)ei = 0. In the same way it follows that
Q(h)e\ = 0 and Q(ii)ei = e and Q(j)e0 = e = Q{j)e\. Now let ??,?? represent
??,?? € 7rfc(X). Then cto + ui is represented by
We just computed Q(io),Q(ii) and Q(j). Since (??,??) ? i0 = a0 and (??,??) ?
ii = ??, it is immediate from these computations that Q(ao,a.i)v = {u;ao)eo +
(v\a\)ei, and so Q(a)v = ((?;?0} + (?;?·1))?, as desired. D
Sullivan Models 173
Lemma 13.11 shows that (—",—) induces a natural linear map
vx : V —> Homs (?.(?'), ?) , ? ·-* (?; ->.
In §15 we shall show that if H*(X;Q) has finite type then this map is a linear
isomorphism.
Finally, recall that m ? : (AV'x,d) —> Apl{X) denotes the minimal Sullivan
model of our simply connected topological space X. On the one hand we have
the Hurewicz homomorphism hurx : ??,(?') —> H,(X:Z). On the other, since
Imd c ?--?"?, division by ?-2?? defines a linear map ? : H+(AVX) —> \'?.
It is immediate from the definition that
[z] G H+(\\\.d)
= (H(mx)[z],hurx{a)) J^.^
Thus the diagram
H+(AVX) H{'2x)-~ Hm(X;
Horn (?,(?'), ?)
commutes, where hur\ is the dual of hurx.
(d) Cell attachments.
Fix a continuous map
a:{Sn,*)—>(X,*), n>l,
into a simply connected topolog'ical space A", representing q € ??(?). The space
A"Ua Dn+1 is the space obtained by attaching an {n + \)-cell to X along a. Now
choose a minimal Sullivan model
mx:(AV,d)-^APL(X).
Our objective is to use this, and the pairing (—; —> : V ? ??,(?') —> A, to
construct a commutative model for A' Ua Dn+1.
If ? = 1 then A" Ua D2 ~ A" V S'2, because ? will be based homotopic to the
constant map. In this case the discussion of wedges in §12(c) shows that A"Ua D2
has a commutative model of the form (??·* ? ku.d) in which deg u = 2, (AV.d)
is a sub cochain algebra, u ¦ A+V = 0 = u2, and du = 0.
For ? > 2 define a commutative cochain algebra (??? © ku,da) by.
• degti = ? + 1.
• AV is a subalgebra and u · A+V = 0 = u2.
• dau = 0 and dav — dv + (v; a)u, ? € V.
174 13 Adjunction spaces, homotopy groups
Note that da? = dz if ? € ?-2F because da is a derivation.
Proposition 13.12 The cochain algebra (AV@Jcu,da) is a commutative model
forXUaDn+1.
proof: We apply Proposition 13.8, noting that Dn+1 = CSn. This gives a com-
mutative model for A' Ua Dn+1 of the form A F x.\w (¦& ? [A+W <8> ?+(?, dt)]),
where (AW,d) is the minimal Sullivan model for Sn (Example 1, §12(a)). The
quasi-isomorphism (AW,d) —> (H(Sn),0) then defines a quasi-isomorphism
AV xaw (Jfc © [A+W ® A+(t,dt)]) ? AF x//(Sn) (?* ? [#+(Sn) ® A+(i,df)D·
These constructions are made using a Sullivan representative c^a : (AF, d) —>¦
(??'?,??) for a. Let ^ be the composite of ?? with m : (AW,d) -%¦ H(Sn) and
define a linear inclusion ? of A F into the fibre product by
?A) = 1 and ?? = (?,^? ® ?), ? G A+V .
Now H+(Sn) = Jk[e], where [e] is dual to the fundamental class [Sn]. Hence
?? = (?,?) if ? € A^2F or if ? € Vfe,fc ? ?. Moreover, for ? e Fn,
?? = (?, (v;a)[e] ® t) .
It follows at once that ? is an algebra morphism and that it can be extended
to a cochain algebra morphism
(AV ® Jfcu, dQ) —* ?V ? wEn ) (A © [H+ En) <8> ?+ (i, dt)] )
by defining u ?—? ( — l)n[e] <8> dt. It is a trivial verification that this is a quasi-
isomorphism. ?
Remark In the situation of Proposition 13.12, consider the commutative square
of continuous maps,
X\JaDn+1 ^ A"
A3.13)
Dn+l " : 5"
This diagram determines the row exact diagram
> APL{X ue ?>n+1, A') ». APL{X UQ Dn+1) > APL(X) > 0
APL(Dn+\Sn) >¦ APL(Dn+1) >APL(Sn) >0
A3 14)
Sullivan Models
175
The proofs of Propositions 13.8 and 13.12 show that this is connected by a chain
of quasi-isomorphisms to the diagram
0
in which:
¦ku·
ku
(AV@ku,dQ)
I
3
i
k ® kw ? kdw
(AT7,·
0
o,
A3.15)
Xu = dw, ?u — dw, ?? = (?; a)w, and (gw, [Sn]} = 1.
(e) The Whitehead product and the quadratic part of the differen-
tial.
Let X be a path connected topological space. The Whitehead product is a
map
[ , ]\V : nk(X) x ??(?) —> nk+n_i{X).
We define it now and then show how, if A' is simply connected, to read it off
from the differential in a minimal Sullivan model.
Recall that in Example 5, §1, homeomorphisms Ik/dlk -^ Sk and dlh+1 -zz±
Sk are specified for k > 1. Regard the first homeomorphism as a continuous
map ak : (Ik,dlk) —> (Sk,*). Thus
akxan:{lk+n,dlh+n,yo) -» {Sk ? Sn,Sh V 5", *) , y0 = A,1,. · ·, 1).
Use the second homeomorphism to identify ak x an L/fc+n a^ a continuous map
The homotopy class [ak,n] € ?·?;+?_1E/: V 5") is called the universal (k,n)-
Whitehead product. (This is actually an abuse of language. The map aktH must be
precomposed with an orientation-preserving rotation sending * to xq. However
any two such relations are based homotopic, so the based homotopy class of the
composite depends only on aktJl.)
When k = ? = 1 the picture
176 13 Adjunction spaces, homotopy groups
identifies [aiti] as the commutator [io]" [ii]—1 [*o][ii]-
Definition The Whitehead product of 70 G 7ik(X) and 71 G ??(?) is the
homotopy class [70,7i]w G Kk+n-i(X) represented by the map
[cccyhv : Sk+n~l -^ Sk V5
where cq : Sk —> X represents 70 and C\ : Sn —> X represents 7X.
Next, consider a minimal Sullivan algebra (AV,d). The restriction of d to V
decomposes as the sum of linear maps a, : V —> Az+lV, i > 1. Each a,· extends
uniquely to a derivation d, of AV. and di increases wordlength by i. Moreover d
decomposes as the sum d = dx + dz H of the derivations di. Clearly, d\ raises
wordlength by 2 and d2 — d\ raises wordlength by at least 3. Since d2 = 0 this
implies d\ = 0; i.e.
• (AV,di) is a minimal Sullivan algebra.
Definition di is called the quadratic part of d.
Recall from §12(b) that the linear part of a morphism ? : (AV, d) —> {AW, d)
of Sullivan algebras is the linear map Q{ip) : V —> W defined by ?? — Q(<p)v G
A-2W. It extends uniquely to a morphism of graded algebras, AQ(<p) : AV —>
AW. If (AV, d) and (AW,d) are minimal then the same calculation as for d\
above shows that (AQ(ip)) d\ = dx (AQ(ip)); i.e.,
• AQ{<p) : (AV, di) —> (AW,di) is a dga morphism between minimal Sullivan
algebras.
Finally, suppose that X is a simply connected topological space, with minimal
Sullivan model
mx :(AVx,d)^APL{X).
Define a trilinear map
by
?,7?> = (^7i>(^7o>+(-l)gdeg'0(y;7o>K7i>, j ?' ,v,
7o» 7i G ?,(?;
Proposition 13.16 The Wliitehead product in ?, (A") is dual to the quadratic
part of the differential of (AVx,d). More precisely,
? G VY ,
(di«i7o,7i> = i-D^-'^hclijw), 70 € irk(X) ,
71 G 7??(?) .
Sullivan Models
177
proof: For the sake of simplicity we denote the universal Whitehead product
[ak,n] simply by a G irk+n-i(Sk V Sn). If c0 : E*,*) —> (X..*) and cx :
(Sn, *) —> (X, *) represent 70 and 7!, then put c = (co:ci) : Sk V 5" —> X, so
that [70,71]w = it«(c)q. Hence
(?;[7?,7?]??) = (Q(c)w;a·).
Moreover, if iQ : Sk —> Sk V Sn and ii : 5" —> Sk V 5" are the inclusions, then
(di«;To,7i> = (dit'iMcHto], ?<#?]> = (diQ(c)v; [io],[»i]> ·
It is thus sufficient to prove the proposition for X = Sk V 5" and 70 = [z'o]*and
7i = [ti]·
For this we require the minimal Sullivan model of 5* V 5", at least up to
degree k + n — 1. First, let m0 : (AW0,d) -%¦ APr,(Sh) and mi : (A\Vi,d) -%¦
APL(Sn) be the minimal Sullivan models of Example 1, §12(a). Thus (AW0,d) =
(A(eo,...),d), (AWi,d) = (A(ei,...),d) and (H(mo)[eo},[Sk}} \= 1 =
(H(mi)[ei],[Sn]). Let p0 = (id,const.) : Sk V 5" -> 5fc and pi = (const., id) :
Sk V 5" —>· 5" denote the projections. Use the construction process of Propo-
sition 12.2 to extend the morphism (???(??)??,?) · (???(??)???) : (?Wo,ci) ®
(???'?, d) —»· ApL (Sk V 5") to a Sullivan model
, y,,y2f... ), d)
i V 5n),
in which: dx = eo <8> ex and degy; > degx, i = 1,2,... . Denote this model
simply by m : (AV,d) —* APL(Sk V 5").
Next write ? = at,„, so that SkxSn = (Sk\zSn)Ua(IkxIn) . The commutative
square
Sk ? Sn = (Sk ? 5") Ua (Ik ? 7") «(Po'Pl) Sk ? 5"
7 X 7" " : ek+n—l
3
is precisely A3.13), where ak and an are the maps defined at the start of this
topic. In particular, Proposition 13.12 asserts that (AV © ku,da) is a commuta-
tive model for Sk x 5", and provides an explicit isomorphism of H{AV ? fcu, dQ)
with77*Efc ? 5"; it).
We shall use this isomorphism to evaluate the classes [eoei] and [u] on a
certain homology class, [z]. The identity map of 7 is a singular 1-simplex l G
Ci G; ?). The chain iuk = EZ{l®---®l) g Ck{Ik;Z) projects to a relative cycle
representing \Ik] ? Hk{Ik,dIk;Z), as was observed in §4(c). Moreover, because
178 13 Adjunction spaces, homotopy groups
at- : dlk —> {pt}, and because C*({pt};Z) = ?-pt, it follows that C*(ak)wic is a
cycle in Ck(Sk\Z). Define a cycle ? G C*(Sk x 5n;Z) by
? = EZ {C*{ak)wk <g> C»(a„)u;„) = C,(ait x a„)tufc+n.
As observed in §4(c), the cycle C,(ak)wk represents [Sk]. Since (§4(b)) EZ
and AW7 induce inverse isomorphisms of homology, a quick computation gives
<[eo ® ei], [*]> = <# (mo)[eo] ® # (m,)[ei], [5*] <8> [5n]> = (-l)*n.
On the other hand, it follows from diagram A3.15) that H*(ak x dn)[u] = ?*?,
where: [u] is regarded as a relative cohomology class in H*(Sk ? Sn, Sk V5n; Je),
? G Hn+k~1(Sn+k~i;]k) is dual to [S"*] and d* is the connecting homor-
phism in cohomology for the pair (Ik ? In,Sn+k~1). Hence
{[u],[z]) = ([u],H.(ak x an)[Ik+n}) = (d*u,[Ik+n}} = (-l)k+n(u,d[Ik+n}) .
But, as observed in §4(c), d[Ik+n] = [5*4""-1]. Thus ([u],[z]) = (-l)A+n.
Since dax = ec,e\ + (x;a)u (cf. §13(d)) it follows that [????] + (s;q)[u] = 0.
Evaluate this equation at [z] to find (-l)kn + (-l)k+n(x;a) = 0: i.e.
In particular it follows that
{dix; [to], [*i]> - (eo ® er, [to], [t"i]> = (-
and
Unless k = ? is even, y*4"" = Jca;, and this completes the proof. In the
remaining case, e'o and e\ both have degree k + n — 1. However poa : Sk+n~1 —>¦
5* factors as S*4""-1 —»¦ 7fc ? /n —». 5fc ? 5n —> Sk, and hence is homotopic
to the constant map. Thus
(e'0;a) = (Q{po)e'o;a) = (e'0;Q(p0)Q(a)[idSn+,-i]) = 0
and
(dieo;[<o],[<i]> = (eg-,[io],[ii]>=0.
The same holds for e[, and so the proposition follows in this case as well. ?
Example 1 The even spheres S2n.
Let ? ? ?2?E2?) be represented by the identity map, and recall that the
minimal Sullivan model of S2n has the form (A(e,e;), de' = e2). It is clear that
(e;a) = 1, and so
{e';[a,a]w) = -(e2;a,a)= -2.
In particular, [?,?]?? is not a torsion class in 7r4n_iE2n). D
Sullivan Models 179
Example 2 S3 V S3 U/ (?>g II D?).
Let ??,?? : S3 —i S3 V 53 denote the inclusions of the left and right hand
spheres, and put a{ = [at·] S ??3E3 V S3). Attach Z>g and Df to S3 V S3
by the maps [ao, [ao>ai]w]w and [??, [ai,ao]w]w The same argument as in
Proposition 13.12 shows that the resulting space has a commutative model of
the form (AV ? Jhuo ? Jku\,D) where uoui = Uq = u\ = uqA+V = Ui A+V" = 0.
Duo —'Du\ = 0, andDv = dv+(v;[ao,[ao,a\]\v]w)uo + (v;[an,[an,ao]w]w)ui.
Of course deg u0 = 8 = degU\.
Now (AV, d) = (A(eo, ei, x, yo, y\, z\, z-z, ¦ ¦. ), d) with
dx = eoei ^yo = eo%, dy\ = e\x
and deg z; > 9, i = 1,2,... . From Proposition 13.16 it follows that Dy0 =
eox + uo, Dy\ = e\X + U\ and D = d on the other basis elements of V.
Moreover, it follows from diagram 13.15 that uq and u\ represent the coho-
mology classes dual to the cells Dq and Df, so that [1], [eo], [ei], [uq] and [ui] is
a basis for H(AV ? Iiuq ? lku\, D). This implies that a quasi-isomorphism
(AV ® kilo + Jkui, D) -=> (A(eo,ei,x)/eoeix, d)
is given by e0 ·->· eo, e\ ·->· ei, s ·->· x, y,· ·->· 0, z,· >-)¦ 0, uo ·->· —eox, andui >-)¦ —e\x.
Thus (A(eo,ei,x)/eoeix, d) is a commutative model for 53 V 53 U (Do LI-0!)·
We may use this to calculate the minimal Sullivan model of 53V53U(D^ IJ Df),
which has the form
(A(eo,ei,x,w,wo,wi,...), dw = e^eix, dwo = eow, dw\ = e\w,... ).
Note that (w;[a.?]w) = 0 for all homotopy classes ? and ? in
?. (S3VS3V(D8IJD?)). D
Exercises
1. Let / : (A',*) -> (Y, *) be a continuous map between simply connected
topological spaces. Determine a Sullivan representative of ?/ : ?? -> ??.
2. Prove that the cofibre of the natural inclusion CP2 c—^CP5 and the space
56V58V510 have the same Sullivan minimal model.
3. Let (AV, d) be the minimal model of S2 V S2. Determine the elements of V-5
which are dual to Whitehead products.
4. Let AT be a simply connected ?-space. Prove that [a,?]w = 0 for every
?
5- Let X be a simply connected space and ? € ??(?), ? G ??(?).
a) Prove that if ? : nq(X) —> irq+i(X) denotes the morphism induced by the
suspension map [S",X] -*¦ [5«+1,??'] then E([a,?]w) = 0.
180
13 Adjunction spaces, homotopy groups
b) Prove that if hurx : ?, (A') -4 Hg(X) denotes the Hurewicz homomorphism
then hur([a,?]w) = 0.
6. Let X be a 1-connected space. Prove that if X is formal then the Hurewicz
homomorphism hurx induces a surjective map ? (?, (A") ® Q)a —>¦ H"(X;Q).
7. Consider the quotient algebra ? = A(x\ ,X2,X3,X4
with deg X!=deg x2=deg x3=3 and deg i4 =5. Prove that the Sullivan minimal
model (AV",d) of (H ,0) is given in low degrees by the following table. (The
reader is invited to complete the table for K4-15.)
y2Xi , 2/3^4
y3X2 ,2/3^3
y 1X3X4
y 2
y
2/i
1X2X3X4
\X0X4 \\X3X4
xxx2 \x\xz 11 3:33:3
V11
???
Vg
V8
Vr
Ve
K3
V,
V,
?;
Assuming that (aV, d) is the minimal model of a space A', express y\, z\ and
u in terms of Whitehead products of A- Define D : Vo © Vx © Vo —> AV by
Dx{ = 0,Dyj = dyj,Dzk = dzk,k ? 1 and Dz\ = y\X\ + X3X4. Prove, by
induction on the lower degree, that D extends to a differential on AV such that
Du = ???? —2/2- Determine the minimal model of (AV, D) in low degrees.
8. Compute the minimal model of the space A' = (S% V Sf) U[a,[a,b}w}w e8 (in
low degrees).
14 Relative Sullivan algebras
In this section the ground ring is an arbitrary field Ik of characteristic zero.
In §12 we showed how to model commutative cochain algebras (C, d) by Sulli-
van algebras, (AV, d). Now any cochain algebra comes equipped with the specific
morphism Iz —> (C, d), 1 ?—>· 1. In this section we introduce relative Sullivan al-
gebras (B ® AV, d) and use them to model general morphisms (B,d) —¦» (C, d)
of commutative cochain algebras. We shall then prove existence and unique-
ness theorems in this setting. This will, in particular, eliminate the hypothesis
H1 = 0 that was frequently supposed in §12.
Definition A relative Sullivan algebra is a commutative cochain algebra of the
form (B ® AV, d) where
• (B, d) = {B ® 1, d) is a sub cochain algebra, and H°(B) = lz.
• V = U V(k), where V@) C V'(l) C · · · is an increasing sequence of
graded subspaces such that
d : V@) —¦> ? and d : V(k) —¦> ? ® AV(k - 1)., k > 1.
The third condition is called the nilpotence condition on d. It can be restated
as follows: if we put V(—1) = 0 and write V{k) = V(k - 1) <g> Vk for some graded
subspace Vk then
? ® AV(k) = [B® AV(k - 1)] ® AVk, and d : Vk —> ? ® AV(k - 1), fc > 0.
Note that we identify ? = B (g) 1 and AV = 1 ® AV. We shall follow this
convention throughout the rest of this monograph. However, while (B. d) is a
sub cochain algebra it will almost always be the case that the differential does
not preserve AV. The sub cochain algebra (B,d) is called the ease algebra of
(B®AV,d).
A Sullivan algebra is just a relative Sullivan algebra with ? = h. On the
other hand if ? : (B, d) —> Ik is any augmentation then applying 3c cEDb — to
(B ® AV, d) yields a Sullivan algebra (AV, d). the Sullivan fibre at ?.
Now. generalizing the Sullivan models of §12, we consider morphisms of com-
mutative cochain algebras
? : (B, d) —> (C, d)
such that H°(B) - k and make the
definition A Sullivan model for v? is a quasi-isomorphism of cochain algebras
m : (B ® AV, d) A (C, d)
182 14 Relative Sullivan algebras
such that (B®AV, d) is a relative Sullivan algebra with base (B, d) and m\B = ?.
If f : X —> Y is a continuous map then a Sullivan model for Api (/) is called
a Sullivan model for /.
In the case of the morphism A* —> (A, d) this definition reduces to the defi-
nition of a Sullivan model of (A,d). As in that case, we shall frequently abuse
language and refer simply to (B <2> AV, d) as the Sullivan model of ?.
Also as in the case of Sullivan algebras, the minimal relative Sullivan algebras
play a distinguished role:
Definition A relative Sullivan algebra (? ® AV.d) is minimal if
Imd C B+ <g> AV + B® A^2V.
A minimal Sullivan model for ? : (B,d) —> (C,d) is a Sullivan model (? ®
AV,d) -=> (C,d) such that (B <g> AV,d) is minimal.
Suppose ? : (B, d) —> (C, d) has a Sullivan model m : (B <8> AV, d) -=» (C, d).
By definition, H°(B) = h and the isomorphism if (m) identifies ?(?) with ?(?),
where ? is the inclusion of ? in B® AV. Since V is concentrated in degrees > 1,
it follows that H°(C) = k and ??(?) is injective.
Conversely, if H°(B) = H°(C) = k and if ??(?) is injective we shall show
that ? has a minimal Sullivan model, determined uniquely up to isomorphism.
This depends on another result we shall also establish: any relative Sullivan
algebra is the tensor product of a contractible Sullivan algebra and a minimal
relative Sullivan algebra with the same base.
The importance of relative Sullivan algebras resides in the fact that they
provide good models for fibrations. Indeed, suppose ? : X —> Y is a fibra-
tion with fibre F and suppose (APL(Y) <g> AV, d) is a relative Sullivan model
for APl{p) : Apl{Y) —¦> APl(X)- In §15 we shall show that if Y is sim-
ply connected and if one of H?{F;k), Hm(Y;k) has finite type then (AV,d) =
&®APL(Y) {Apl{Y) <8>AV, d) is a Sullivan model for F. It is from this that we
deduce that the morphism defined in §13(c) is an isomorphism:
for minimal Sullivan models (AV,d) of suitable topological spaces X.
This section is organized into the following topics:
(a) The semifree property, existence of models and homotopy.
(b) Minimal Sullivan models.
(a) The semifree property, existence of models and homotopy.
Let (B ® AV,d) be a relative Sullivan algebra. Multiplication by ? makes
(B (g> AV,d) into a left (B, d)-modu\e (cf. §6) and we shall make frequent use of
Sullivan Models 183
Lemma 14.1 (? ® AV, d) is (?, d)-semifree.
proof: Write V = \J V(k) as in the definition, and set V(-l) = 0. Write
V(k) = V(k - 1) © Vk, and simplify notation by writing ? <g> AV(k) = B{k).
Then B(k) = B(k - 1) ® AV* and d:Vk-+ B(k - 1). Hence
Assume by induction that (B(k - l),d) is (B, d)-semifree. Then this equation
identifies the quotient on the left as (B, d)-semifree for each ? > 1. It follows
from Lemma 6.3 that each (B(k),d) and (B(k),d) / (B(k - l),d) are (?,«*)-
semifree. Now a second application of Lemma 6.3 shows that (B cg> AV, d) =
\Jk (B(k), d) is (B, d)-semifree too. ?
A second useful fact is the 'preservation under pushout' of relative Sullivan
algebras. Suppose (B <g> AV, d) is a relative Sullivan algebra and ? : (B, d) —>
(?', d) is a morphism of commutative cochain algebras with H°(B') = h. Then,
immediately from the definition, the cochain algebra
(B1, d) ®(B,d) (B ® AV, d) = (B' ® AV, d)
is a relative Sullivan algebra with base algebra (B',d). It is called the pushout
of (B ® AV, d) along -?.
Notice that associated with the pushout is the commutative diagram of cochain
algebra morphisms
(B,d) (B®AV,d)
(B',d) (B'®AV,d).
To see that -? ig> id commutes with the differentials, identify ? ® AV = ? ®?
(B ® AV) and observe that '
? ® idjiv =?®? {idB®\v) :B®B{B® AV) —> B' ®B (B ® AV).
Now Proposition 6.7(iii) asserts that —®b{B ® AV, d) preserves quasi-isomorphisms
because (B <S> AV, d) is (B, d)-semifree. There follows
Lemma 14.2 If ? is a quasi-isomorphism so is ? Cg> id : (B <S> AV, d) —>
{B'®AV,d). ' D
We can now establish the existence of Sullivan models for morphisms:
Proposition 14.3 A morphism ? : (B,d) —> (C,d) of commutative cochain
algebras has a Sullivan model if H°(B) = k = H°(C) and ??{?) is injective.
184
14 Relative Sullivan algebras
proof: Choose a graded subspace Bx C ? so that
(BrH = A, {B^1 ®d(B°) = B1 and (Bj)" = B'\ ? > 2.
Clearly (i?i, d) is a sub cochain algebra and the inclusion ? : (??, d) —¦» (?, d)
is a quasi-isomorphism. In particular the restriction ?\ : (B\,d) —l· (C,d) of ?
satisfies: ^(??) is injective.
Now because (??)° = Ik the argument of Proposition 12.1 applies verbatim to
show the existence of a Sullivan model mi : (B\ ® AV, d) -^> (C, d) for c?x. Thus
a commutative diagram of cochain algebra morphisms is given by
{B,d)®{Bud){Bx®AV.,d)
AV, d)
in which ,7B) = 1 ®?? a.
This may be rewritten as
{B®A\'\d)
Lemma 14,2 and the preceding remarks show that ?
and (B ® AV, d) is a Sullivan algebra; hence m :
Sullivan model for ?.
is a quasi-isomorphism
AV,d) -^ (C,d) is a
?
Finally, we extend the lifting lemma to the relative case and define relative
homotopy. To begin, suppose given a commutative diagram of morphisms of
commutative cochain algebras
(B,d)
(? ® AV, d)
(A,d)
(C,d)
in which i is the base inclusion of a relative Sullivan algebra and 77 is a surjective
quasi-isomorphism. An argument identical to that of Lemma 12.4 establishes
Lemma 14.4 There is a morphism ? : (B(&AV,d)
(io extends a) and ?? = ? (? lifts ?).
(A,d) such that ?? = ?
0
Sullivan Models 185
We come now to the notation of relative homotopy. Suppose
??,??: (B®AV,d) —)· (A,d)
are two morphisms of commutative cochain algebras, in which (B ® AV, d) is
a relative Sullivan algebra and ?? and ?? restrict to the same morphism ? :
(B,d) —>· (A,d). Generalizing the definition in §12(b) we have
Definition ?? and ?? are homotopic rel ? (?0 ~ ?? rel ?) if there is a mor-
phism
? : (? ® AK d) —> (.4, d) ® A(i, dt)
such that (????)? = ??, (id ??)? = ?? and ?(?) = ? F) ® 1, b e B. The
morphism ? is called a relative homotopy from ?? to ??,
The identical argument of-Proposition 12.7 shows that homotopy rel ? is an
equivalence relation in the set of morphisms ? : (? ® AV, d) —> (A, d) that
restrict to a given ? ·, (B, d) —¦» (.4,ef). The homotopy class of ? is denoted by
[?] and the set of homotopy classes is denoted by [(B ® AV, d), (A. d)]a.
Notice that ? ; (B, d) —¦» (.4, d) makes (.4, d) into a (B, d)-module via b· a =
a(b)a. Now suppose ??·,?\ '¦ (B ® AV, d) —¦» (-4, d) both restrict to ?.
Lemma 14.5 -??c/? ~ ^? rel i? ?/ien ??~^>\ = hd+dh, where h : B®AV —>· .4
zs ? B-linear map of degree —1. In particular, ?(?0) = ?(??).
proof: Let ? : (? ® AV,d) —¦> (.4, d) ® A(i,di) be a homotopy rel ? from <^0
to ??. As in the proof of Proposition 12.8, define h : ? ® AK —¦> .4 by
?B) = pot*) + (^ (z) - c^oW) ? + (-l)degi/iB)cii + ?,
where ? € A®(I + dI), I C A(f) denoting the ideal generated by i(l - i). Then,
because ?? = ??, we obtain ?0 — ?? = dh + hd. Moreover, since ? restricts to
? in ? it follows that ?(??) = ?(?)?(?), be ?. Hence h{bz) = (-l)dezbb-h(z);
i.e., h is i?-linear. D
Finally, suppose that
(B,d) (A,d)
(? ® ??, d) (C, d)
is a commutative square of morphisms of commutative cochain algebra, in which
• i is the base inclusion of a relative Sullivan algebra, and
• ? is a quasi-isomorphism.
186 14 Relative Sullivan algebras
Note that we do not require that ? be surjective.
The proof of Proposition 12.9 also goes over verbatim to give
Proposition 14.6 (Lifting lemma) There is a unique homotopy class rel ? of
morphisms ? : (? <%> AV, d) —¦» (A,d) such that
ip\R=a and ??^-???? ?.
(b) Minimal Sullivan models.
Here we establish three basic results.
• Any relative Sullivan algebra is the tensor product of a minimal relative
Sullivan algebra and a contractible algebra (Theorem 14-9).
• A quasi-isomorphism between minimal relative Sullivan algebras is an iso-
morphism, provided it restricts to an isomorphism of the base algebras
(Theorem I4.II).
• Minimal Sullivan models of cochain algebras and of morphisms exist, and
are unique up to isomorphism (Theorem 14-12).
For ordinary Sullivan algebras these results extend Proposition 12.2 and several
results in §12(c) to the case where Hl{—) is not necessarily zero.
Before beginning the proofs, we make two small but useful observations. Con-
sider first an arbitrary commutative graded algebra of the form ? = Jk&lB'}^,
and a graded vector space V = {V'}i>i. Let W C (B ® AV)+ be a graded sub-
space such that
(B ® AV)+ = W © A^-V ? (B+ ® AV).
The inclusions of ? and W extend uniquely to a morphism ? : ? <g> AW —>
? ® AV of graded algebras.
Lemma 14.7 The morphism a : B ® AW —¦» ? <S> AV is an isomorphism.
proof: It follows from the hypothesis that Vn C Wn + ? ® AV<n, n > 1, and
an obvious induction then gives that ? is surjective. Choose ? : V —¦> ? <S> AW
so that ?? = id, and extend id? and a to the morphism r = id ? ·? ·' B®AV —>
? 0 AW. Then ?? = id.
Now suppose by induction that ? and r restrict to inverse isomorphisms be-
tween B®AW<n and B®AV<n. Let w € Wn. Then tw = w'+ u with w' 6 Wn
and «e?+® AW<n. Hence w = ???? = aw' + au = w' + au. In other words,
w - w' = au e W ? (B+ ® AV<n) = 0 .
Sullivan Models 187
By induction, ? is injective in B<8)AV<n. Hence w = w' and u = 0: i.e., tw — w.
This shows that ?? = id in Wn: which closes the induction. ?
Next, suppose (B ® AV, d) is any relative Sullivan algebra. Choose a graded
vector space fi,c?so that {??)° = k, (??I ? d(B°) = B1 and {??? = B'\
i > 2. Then B\ is preserved by d, (Bud) is a sub cochain algebra and the
inclusion in (B, d) is a quasi-isomorphism.
Lemma 14.8 T/iere zs ? relative Sullivan algebra {B\ ® AV, d') and an isomor-
phism,
?:{?® AV, d') = (B, d) ®[Bl,d) (#i ® AV, d') A (B ® AV, d),
restricting to the identity in B.
proof: Write F = (J V(jfc) with
AV(Jfe), d) = (? ® AV*(A: - 1) ® AV'fc, d)
and d : V* —¦> ? ® AV(A: — 1). Assume by induction we have constructed
(Bi ®AV(k- l),d') and
- 1), d') A (? ® AV(Jb - 1), d).
Lemma 14.2, applied to the inclusion (??,?) —>· (B,d) shows that the inclusion
(?i (g) AV(a; - l),d') —¦> (? ® AV(A; — l),d') is a quasi-isomorphism. Hence if
{va} is a basis of V^ there are d'-cocj-cles za G ?? ® ? F (A: - 1) and elements
ya e B ® AV{k — 1) such that di>Q = ?B? + d'ya). Extend d' and ? to Vit by
setting d'va = za and ??? = va - ayQ.
By construction, ? is a cochain algebra morphism. By induction, it restricts to
an isomorphism of B®AV(k— 1) onto itself. Denote the inverse of the restriction
by r, extend rto?® AV(k) by setting ??? ~ va + ya, and verify that r and ?
are inverse isomorphisms of B (g) AV(k). D
Theorem 14.9 Let (B<g>AV, d) be a relative Sullivan algebra. Then the identity
of ? extends to an isomorphism of cochain algebras,
(B ® AW, d') ® (A(U ? dU),d) ? (? ® AV, d),
m ly/izc/i (?® AH7,d') ? ? minimal relative Sullivan algebra and (A(U ? dU),d)
is contractible.
proof: First consider the case ? = k © {?'};>!. The decomposition
(B ® AV)+ = (B+ ® AV) ? A^2V ? V
Igg 14 Relative Sullivan algebras
defines a differential d0 : V —¦» Vr by the condition dv-dov G (B+®AV)©A-2V,
y c. y? Write V as the increasing union of graded subspaces V(k) such that
d : vr(fc) —» ? ® AV(k - 1) and note that
d - d0 : V(fc)n —» ? ® ? (V(Jfe - l)-n) .
Choose an increasing sequence D"@) C U(l) C · · · C V of graded subspaces
such that
U(k) e (kerdo ? V(fc)) = V(k).
(Since t/(fc - 1) ? (kerd0 ? V(fc)) = 0. i/(fc - 1) can be enlarged to the direct
summand U(k).) Set U = \Jk U(k). Then U ? kerdo = V and so we may write
V = t/ ? d0U ? IK d0 : U A do I/, d0 : W —>· 0.
This construction has the following useful property: for elements u, u\ G U
and -u; G H7,
u\ + dou + w G K(fe) => m G V(k). A4.10)
Indeed, we may write u\ + dou + w = u' + z' with u' G U(k) and z' G kerdo-
Thus u? — u' = z' — dou - w G U ? kerd0 = 0, and u\ = u'.
Now consider the subspace U ? dU ? W C ? ® AV. Apply Lemma 14.7 to
conclude that this inclusion, together with ???-, extends to an isomorphism of
graded algebras,
? : ? (g) AU ® Ad?7 ® AH7 A?® AV.
Denote the contractible algebra AU ® Adi7 simply by ?, and let d = ?~???
be the induced differential in ? <g> ?" ® AH7. Use ? to identify the two cochain
algebras and so regard I7 as a subspace ?? ? <& ? <E> AW.
Our next step is to exhibit the inclusion
(B, d) ® (?, d) —> (B ® ? ® AW, d)
as the inclusion of a relative Sullivan algebra. Define an increasing sequence of
graded subspaces W@) C W(l) c · - - C W by W@) = {w e W \ dw e ? <S> E}
and W(t) = {w e\V \dw e B®E® AW(i - 1)}, ? > 1. Set ? = \J( W{i). It
is enough to show ? = W.
Assume V<n C ? ® ? ® AZ. We show first by induction on k that
V(k)n CB&E&AZ.
I21 deed if u G V@)" we have dv G ?, so that do ? = 0 &?? ? = dou + w, some
u G Un~x, w G II7". As observed above, (do - d)u G ? ® AV<n, and hence
(do - d)tx G ? ®E® AZ. It follows that
d(dou) = d(do - d)u G ? ® ? ® AZ.
Sullivan Models 189
But dv G B and so dw = dv - dd^u G B ® ? ® AZ. In particular, we must have
dw 6 B®E®AW(?), some ?, and so w G IF(?+ 1). On the other hand, we have
just seen that (do-d)u C B®E®AZ. Since du ? ? by definition, it follows that
dou? B®E®AZ. Thus ? -dou + w 6 5® ?® AZ; i.e., F@)n C B®E®AZ.
Suppose V(k— 1)" C i?<g>.E® AZ. Let ? 6 V(A:)n and write ? — U\ +dou + w,
with ui € Un, u e Un~l and ? G TV". As observed in A4.10), then ux G lr(fc).
Hence (d - do)ux C. ? ® i\V{k - \)^n C ? ® ? ® AZ. The same argument as
just above for k = 0 shows that (d - do)dou = d(d0 - d)u S ? ® ? ® AZ. Hence
dw = (d- do)w ? ? ® ? ® AZ
and so, as in the case k — 0, it follows that ? 6 Z. As in that case, dou e
B&E&AZ, while Ux e ? by definition. This shows that V(k)n C B®E®AZ.
It follows by induction on k that Vn C B®E®AZ, and so a second induction
on ? shows that V C ? ® ? ® AZ. This implies that ? ® ? ® AZ is all of
? ® E® AW, and so^= f-F, as desired.
Finally, let ? : ? —-> Jfc be the augmentation. Then id ·? : (B,d) «8> (?,d) —->
(B,d) is a quasi-isomorphism. Hence it defines a suijective quasi-isomorphism
id -? ® id : (? ® ? ® AW, d) —-*· (? ® AH': d')>
as described in Lemma 14.2.
In particular, (B ® AW, d') is a relative Sullivan algebra and, since d : II' —->¦
B+®E® AW + {B®E® AW)+ ¦ (? ® ? ® AW)+, it follows that d' : W —>·
5+® ??^??^??; i.e., (B®AW,d') is minimal. Apply the Lifting lemma 14.4
to extend id ? to a morphism
? : (? ® AW, d') —> (B ® ? ® AIF, d)
such that (id -c ® id)^ = id.
Then ?-w—w G ?®J5+<8>AVF. This implies (Lemma 14.7) that an isomorphism
(B ® AW, d') ® (A(U © dU)) ? (? ® AV, d)
is given by ? ® ? ·->¦ ?(<^? · ?). This completes the proof of.the theorem in the
case B° = k.
Finally, suppose (B ® AV, d) is a general relative Sullivan algebra. Write
(B<S>AV,d) = (fi,d) ®(Blid) Ei ® AV",d), as in Lemma 14.8, with {B^0 = k.
By what we have just proved, (Bi ®AV,d) s (?? ®AW,d')®A(U@dU). Thus
(? ® AV, d)9i(B® AW, d') ® A(U ? dU). D
Next, consider a cochain algebra quasi-isomorphism
? : (?' ® AV',d) ? (? ® AV, d)
between minimal relative Sullivan algebras.
190 14 Relative Sullivan algebras
Theorem 14.11 If ? restricts to an isomorphism t)q : B' —*¦ ? then ? itself
is an isomorphism.
proof: Consider the diagram
(B' ®AV',d)
(B,d) (B®AV,d)
We apply an argument of Gomez-Tato [65] to extend ??? to a morphism 7 :
(B <g> AV, d) —¦» (B' ® AV, d) such that 777 = id.
By the very definition of minimality, d : V —¦» B+ ® AV ? A^2V. Thus if
F = UA, v(fc) with F(Jfe) = V(k -1) ? Vfc and d : Vk —¦> ? ® AF(jfc -1) it follows
that d : V? —¦> 5 ® AV<n ® A (V(k - 1)"). Thus to construct 7 it is enough to
assume it has been defined in .4 = ? ® AV<n ® A (V(fc — 1)") and to extend it
to A®AV?.
Observe that ? factors to give a quasi-isomorphism of cochain complexes,
B' ® AV ? B®AV
(we are dividing by graded subspaces, not ideals). The cochain complex ? ®
AVI A contains no elements of degree ? — 1, and hence no coboundaries of degree
n. Thus every cocycle of degree n is the image, under 77, of a cocycle of degree
? in (B' ® AV')/j(A). In particular, if {uQ} is a basis of Vfcn then there are
elements xa G ?' ® AV' and elements aQ,a^ G ? such that
rya;Q = uQ + aa and dxa = 7(a'a).
Hence dva = ??(?? — ???) = a'a — daa. Extend 7 to V? by putting ??? =
?? — ??<*·
This completes the construction of 7. Since 7/7 = id, 7 is also a quasi-
isomorphism. Now the same argument applied to 7 gives a morphism ? :
(?' <8) AV',d) —¦> (B (g) AV,d) such that 7? = z'd. Thus 7 is both injective
and surjective; i.e., it is an isomorphism. Since 7/7 = id, ? is the isomorphism
7. D
Finally, suppose
?: (B,d) —)· (C,d)
is a morphism of commutative cochain algebras such that H°{B) = h = H°(C)
and ??{?) is injective.
Sullivan Models 191
Theorem 14.12 The morphism ? has a minimal Sullivan model
m:(B®AV,d) -=>(C,d).
If m! : (B <g>AV,ef) -^» (C,d) is a second minimal Sullivan model for ? then
there is an isomorphism
a:(B® AV, d) A (B ® AV', d)
restricting to id?, and such that m'a ~ mrel B.
proof: In Proposition 14.3 we showed ? had a Sullivan model, and in Theo-
rem 14.9 we showed that this is the tensor product of a contractible algebra and
a minimal relative Sullivan algebra. Thus ? has a minimal Sullivan model.
Given two such models we may apply Proposition 14.6 to the diagram
(B,d) (B®AV',d)
(B®AV,d) = - (C,d)
to extend id? to a morphism ? : (? <g> AV, d) —¦» (? <g> AV, d) such that m'a ~
mrel B. Now Theorem 14.11 asserts that a is an isomorphism. D
Corollary Any commutative cochain algebra (A, d) satisfying H°(A) = .& and
any path connected topological space X have a unique minimal Sullivan model.
Finally, let ? : (AV, d) —y (AW, d) be an arbitrary morphism between Sullivan
algebras. Recall that d0 : V—> V and do : W —> W denote the linear parts of
the differentials (§12(a)) and that the linear part of ? is a morphism of complexes
Q(<p):(V,do)->(W,do).
Proposition 14.13 If ? : (AV,d) —> (AW,d) is a morphism of Sullivan alge-
bras then ? is a quasi-isomorphism if and only if H(Q(ip)) is an isomorphism.
proof: ' WriteJAV, d) = (AV, d) ® (E, d) and (AW, d) = (AW, d) ® (F, d) with
(AV, d) and (AW, d) minimal Sullivan algebras and (E, d) and (F, d) contractible
Sullivan algebras. Let ? be the composite (AV, d) —> (AV, d) -A (AW, d) —t
(AW,d). Then we may identify ?(?) = ?(?) and H(Q(tp)) = Q(^). But by
Theorem 14.11, ? is a quasi-isomorphism if and only if it is an isomorphism. If -0
is an isomorphism so is B(?). Conversely, suppose <^(?) is an isomorphism, then
¦? induces isomorphisms A^mV/A>mV -=> A^mW/A>mW. By induction it in-
duces isomorphisms iW/A>mV A AW/A>mW. Since (AV)k = (AV/A>mV)k
for m > k it follows that ? is an isomorphism. D
192 14 Relative Sullivan algebras
Example 1 The acyclic closure, (B®AV,d).
Suppose (B,d) is an augmented commutative cochain algebra such that
H°(B) = k and Hl{B) - 0. A minimal Sullivan model (B <g> AV,d) -=> Jc
for the augmentation ? : (B,d) —> k is called an acyclic closure for (?,d). We
show that the quotient differential d in AV is zero.
Form the relative Sullivan algebra (B ® AV ®B ? ® AV, d) = E®AV®AV, d).
The inclusions of the left and right tensorands are the base inclusions ??,?? :
(? ® AV, d) —> (? ® ? F ® AF, d) of relative Sullivan algebras. Hence ? ®\0 -
and ?<8>?? ~ are surjective quasi-isomorphisms from (??®AV®AV, d) to (AV, d).
Let ? : (AV, d) -=* (B <8> AV ® AV', d) be a quasi-isomorphism such that {e ®Aj
—) ? a = id (Lemma 12.4). Then (? ®a0 -) ? ? is a quasi-isomorphism of the
minimal Sullivan algebra (AV, d). Hence (Theorem 14.11) it is an isomorphism.
Denote by ? the inverse isomorphism, and consider the composite morphism
? : (AV,d) A (?®AV®AV,d) g®'d®id) (AV,d)®(AV,d) ^^4 (AV,d)®(AV,d) .
By construction, for ? ? A+V we have
?? = ?®\-{-?+1®?, some ? G ?+ V ® A+V.
Hence for any cocycle z,
?(?)[?] - [?] ® 1 - 1 ® [z]
In Example 3, §12(a), we showed that a certain algebra H*(X; ]k) was a free
graded commutative algebra using by the existence of a morphism H*(X; 3c) —>¦
H*(X; k) ®H*(X; k) satisfying this condition. That argument now shows that
H(AV, d) is a free graded commutative algebra. AW. Define a quasi-isomorphism
? : {AW, 0) -=> (AV, d) by sending a basis of W to representing cocycles in AV.
Then ? is an isomorphism (Theorem 14.11). D
Example 2 A commutative model for a Sullivan fibre.
Let ? : {B,d) —> (-4,d) be a morphism of commutative cochain algebras both
of which satisfy H°{-) = k and &{—) = 0. Extend ? to a minimal Sullivan
model m : {B ® AW, d) -?» (A, d) and recall that (AW, d) = k ®B {B ® AW, d)
is the Sullivan fibre of ? at an augmentation ? : ? —> le.
We construct a commutative model for (AW,d) as follows. Let (?®AV,d) be
an acyclic closure for {B,d). Since — ®# {M,d) preserves quasi-isomorphisms
for any (?,d)-semifree module {M,d) — cf. Proposition 6.7 — it follows that
{A ® AV, d) ^HL (jB ? AV) ®B {B 0 AW) -^4 (AW, d)
are quasi-isomorphisms; i.e.
(A ® AV, d) = ,4 ®b {? ® ?V, d)
Sullivan Models 193
is a commutative model for the Sullivan fibre (AW, d). ?
Exercises
1. Let ? : (B<2)AV,d) ->· (B®AW,d) be a morphism of relative Sullivan algebras
which restricts to the identity on ? and let (B®AW, d) ^-T1 (.4, d) ? (.4', d') be
morphisms of commutative differential graded algebras. Assume that ? and ?
are quasi-isomorphisms and that ?? , ?\ restrict to the same morphism (B. d) —>·
{A,d). Prove that the following assertions are equivalent: (i) v?o ~ 9i rel B, (ii)
??$ ~ ?^?? rel B, (iii) ??0 ~ ??\ rel B.
2. Let (AV,d) be a Sullivan minimal algebra.
a) Fix an automorphism ? of (AV,d) such that for each x G (AV,d) there
is some ? (depending on x) such that (? — idAv)n(x) = 0. Prove that ? =
(? - idAy)n is a derivation of (AV,d) , homogeneous of degree 0
?—' ?
k-\
which commutes with d and that for each x G (AV. d) there is some ? (depending
on x) such that ??(?) = 0.
OO .
b) Prove that ? = - Y^ —?? is a linear map which commutes with d
and that ?? = ?? = ? — id.
c) Prove that (Au ® AV. D) with u of degree 1 and Dv = u ® #(?) + 1 ® dw, is
a minimal model of the kernel of e.\ · id — e0 · ? ¦ A(t, dt) ® (AV, d) ->· (AV. d).
3. Let (? ® AV, (i) be a relative Sullivan algebra and consider the contractible
cochain algebra E(sV) = AsV <g> AV where V1 = V\
a) Prove that (B ® AV. ii) ® 5(sV) = (? ® A(V ? sV @ V), D) is a relative
Sullivan model.
b) Prove that the natural inclusion ?0 : (B®AV,d) c—>(B®A(V@sV@V),D)
is a quasi-isomorphism.
c) Consider the degree -1 derivation S defined in (B ® A(V © sV ? V),D) by
S{x) = sx,x GV and S{B) = S{sV) = S{V) = 0. Prove that ? = SD + DS
~ ?n
is a degree 0 derivation and that e6 — > —- is an automorphism of differential
. n!
n=0
graded algebras.
OO Qj
d) Prove that ?? = eoAo satisfies ?? (?) = ? + ? + V] — —E ? d(x)).
i=o (^ + 1)!
e) Let <^o , ?? : (? ® AV. d)^>(A. dA) be morphisms of commutative differential
graded algebras which restrict to the same morphism (B, d) ->· (.4, dA). We write
/ « <7 if there exists a morphism of differential graded algebras ? : (? ? A(V ®
sV ? V),Z)) ->· (.4,6?^) such that ??? = (/?o and ??? = ??. Prove that « is an
equivalence relation and that ?? ~ ?\ implies that ?(?0) = ?{??).
194 14 Relative Sulliva
f) Assume ? — Ik — H°(A). Prove that ?0 m ?? if and only if ?0
this result extend to the case H°(B) = Ik = ?°(?)?
15 Fibrations, homotopy groups and Lie group
actions
In this section the ground ring is an arbitrary field fc of characteristic zero.
In this section we see how relative Sullivan algebras model fibrations. In par-
ticular, if / : ?" —> Y is a continuous map with homotopy fibre F we construct a
Sullivan model for F directly from the morphism Api(f): Apl(Y) —> Apl(X),
provided Y is simply connected with rational homology of finite type.
We use this to construct Sullivan models for many more interesting spaces.
We are also able, at last, to establish the isomorphism
(promised in §12 and §13(c)) between the generators of a minimal model and
the dual of the homotopy groups.
Finally we apply Sullivan models to the study of principal bundles and group
actions of a path connected topological group G. Our main focus is on the case
when H*.(G;]k) is finite dimensional, which includes all connected Lie groups.
In particular, we use Milnor's universal bundle (§2) to obtain a simple form for
the Sullivan model of any principal bundle. We also consider models for group
actions and see, for example, rational homotopy reasons why spaces may not
support free actions of groups such as 53 x SUC).
Much of the material was originally developed by H. Cartan, Koszul and Weil
in the context of smooth principal bundles, with the aid of principal connections
and the curvature tensor. This was described in three lectures [33] [34] [102]
given in Brussels in 1949 and provided one of the major clues that led to Sul-
livan's introduction of minimal models. Indeed, the main result announced in
Koszul's. lecture is the construction of (what we now call) a Sullivan model for a
homogeneous space.
This section is organized into the following topics:
(a) Models of fibrations.
(b) Loops on spheres, Eilenberg-MacLane spaces and spherical fibrations.
(c) Pullbacks and maps of fibrations.
(d) Homotopy groups.
(e) The long exact homotopy sequence.
(f) Principal bundles, homogeneous spaces and Lie group actions.
(a) Models of fibrations.
Consider a Serre fibration of path connected spaces
? : X —> Y,
196 15 Fibrations, homotopy groups and Lie group actions
whose fibres are also path connected. Let j : F —> X be the inclusion of the
fibre at y0 6 Y· Then APl converts the diagram
? _i_4 ? ApL(F) 4^ APL{X)
to I Mpi.(p) A5.1)
yo >Y A i—-—APL(Y) .
where ? is the augmentation corresponding to yo-
Observe that H1 (Apl(p)) is injective. Indeed, since F is path connected it
follows from the long exact homotopy sequence that ??? (?) is surjective (Propo-
sition 2.2). Hence H\{p:Z) is surjective (Theorem 4.19). But
Hi A'; k) = Hi (Y; Q) <8>q k = Hi {Y; Z) ®z k ,
and so Hi{p\h) is also surjective. Thus the dual map, ?1 (APi(p)) = Hl{p;h)
is injective (Proposition 5.3(i)).
Since H1 (APl(p)) is injective, Proposition 14.3 asserts the existence of a
Sullivan model for p,
m : (APL(Y) ® XV,d) -^ APL{X).
(In fact (Theorem 14.12) there is even a minimal Sullivan model for p.)
The augmentation ? : ???(?) —y h defines a quotient Sullivan algebra
(AV,d) = k ®apl(y) {Apl{Y) ® AF,d),
which is called the fibre of the model at yo-
Since APl(j)APl(p) reduces to ? in Apl(Y), APL(j)m factors over ? ¦ id to
give the commutative diagram of cochain algebra morphisms
APL{F) . AplU) APL{X)
A5.2)
(AV,d) ' ^.d (APL(Y)®AV,d)
We show now that, under mild hypotheses, m is a quasi-isomorphism. Thus in
this case m : (AV, d) -=> APl (F) is a Sullivan model for F: the fibre of a model
is a model of the fibre.
Theorem 15.3 Suppose Y is simply connected and one of the graded spaces
H*(Y;k), Hm{F;k) has finite type. Then
m: (AV,5) —> APL(F)
is a auasi-isomorphism.
Sullivan Models
197
proof: Suppose first that ? is a fibration. We wish to apply Theorem 7.10, with
diagram A-5.2) corresponding to diagram G.9). For this we need to verify two
things: first, m has to be an .4p?,(F)-semifree resolution and second, diagram
A5.1) has to be weakly equivalent to the corresponding diagram with C*( —)
replacing Api(-). But the first assertion is just Lemma 14.1 and the second
follows from the natural cochain algebra quasi-isomorphisms C*(—) -^ · <^-
Apl(-) of Corollary 10.10. Thus we may apply Theorem 7.10 and it asserts
precisely that mis a quasi-isomorphism.
Now suppose only that p. is a Serre fibration. In the diagram constructed in
§2(c),
X
Y
X ? ? MY
, ?? = (?, const, path at px),
? is a map from a Serre fibration to a fibration. Since ? is a homotopy equiva-
lence it restricts to a weak homotopy equivalence ? : F -=·» ? ? ? ?? (Proposi-
tion 2.5(?)).
Moreover, .4??,(?) is asurjective quasi-isomorphism. Apply the Lifting lemma
14.4 to the diagram
APL(Y) ApL(q\ APL(X xY MY)
ApL(X)
(APL(Y)®AV,d)
Apl(X)
to construct the quasi-isomorphism ? extending Api{q). Then ? : (AV,d) —>
Apl(X xy PY) is a quasi-isomorphism by the argument for fibrations, and m
is the quasi-isomorphism Apl(X)u. ?
Remark In [82] the theorem is proved under the weaker hypothesis that ?? {?)
acts locally nilpotently in each Hi(F; Jk). It is in fact possible to show that this
hypothesis and the hypothesis that one of H,{Y\ Je), H*(F; le) has finite type
are both necessary for the conclusion of the theorem to hold. ?
More generally, suppose that
f:X
Y
is an arbitrary continuous map from a path connected topological space X to a
simply connected topological space Y. Suppose further that H*(Y;lk) has finite
type. Since Y is simply connected if1 (!';.&) = 0 and H1 (APL(f)) is injective.
Thus / has a Sullivan model
m : (APL(Y) ® AV,d) A APL(X),
198 15 Fibrations, homotopy groups and Lie group action;
as above. In this case the argument in the proof of Theorem 15.3 shows that th<
fibre (AV,d) at yo is a Sullivan model of the komotopy fibre of f.
Return to the situation of the Serre fibration ? : X —> Y with which thi;
topic began, and recall the notation of A5.1). Theorem 15.3 establishes a fun
damental property for relative Sullivan models (Apl(Y) ®AV,d) -^ Apl(X)
It is, however, often useful to replace ApL(Y) by a Sullivan model, and this i:
done as follows. ^
Choose a Sullivan model my : (AVy,d) -^> Apl(Y). Since Vy = {VY}i>:
by definition, there is a unique augmentation ? : (AVy,d) —> k. Construct ?
commutative diagram of cochain algebra morphisms
APL(Y) ApL(p] > APL(X) AplU) > APL(F)
A5.<
(AVy,d) (AVy®AV,d) g .d » (AV,d)
by requiring that
• i is the inclusion of a relative Sullivan algebra, and
• m is a Sullivan model for the composite ApL{p)my.
Then Apl(j)tti factors over ? ¦ id to yield m.
As in Theorem 15.3, we now restrict to the case that Y is simply connectee
and one of H*(F;Jk), H*(Y;]k) has finite type. With these hypotheses we have
Proposition 15.5 The three morphisms my, m and m in A5.4) are a^ Sul-
livan models.
proof: (i) The morphism my. This is a Sullivan model by hypothesis.
(ii) The morphism m. This is a quasi-isomorphism by construction
Thus we have only to exhibit (AVy <g> AV. ci) as a Sullivan algebra. Put W =
Vy © V and define an increasing sequence of subspaces 0 = W(—l) C W@) C
• · · W by setting W{i + 1) = {w € W | dw 6 AW(?)}. It suffices to show that
W = \JtW(?).
Now Vy = Ufc VY(k) and V = \Jk V(k) with d : Vy(k) —> AVY(k - 1) and
d : V(k) —> AVy ®AV(fc-l). Thus VY{k) c W(k). If V(k-l) c {J(W{i) and
? e V(k) then since dv ? AVY <8> AV(k — 1), dv is in some AW(t). Hence ? is in
some W(e+l) and V(k) C \JW{i) as well.
(iii) The morphism m. Write
APL{Y) ®(Avv,d) (AVV ® AV,d) = (APL(Y) ® AV,d) .
This is a relative Sullivan algebra, and m factors as
(AVy®AV,d) ^^ {ApL(Y)®AV,d) ^PL(p)m) APL(X).
Sullivan Models 199
But my.<8> id is a quasi-isomorphism (Lemma 14.2). Hence so is Api(p) ¦ m.
Now apply Theorem 15.3. ?
Since the morphism ApL(p)my has a minimal Sullivan model (Theorem 14.12)
the relative Sullivan algebra (AVy,d) —> (AVy <8> AV,d) may be taken to be
minimal. In this case (AV,d) is minimal and m : (AV, d) -=» Apl(F) is the
minimal· model of F. Thus Proposition 15.5 has the:
Corollary Suppose (AVy,d) is a Sullivan model for Y and (AV, d) is the mini-
mal Sullivan model for F. Then X has a Sullivan model of the form (AVy<g>AV, d)
in which (AVy,d) is a sub cochain algebra and dv — dv G A+Vy <g> AV", ? G V.D
Note: (AVy <8> AV, d) is minimal as a relative Sullivan algebra, but it is easy to
make examples in which it is not minimal as a Sullivan algebra.
Proposition 15.5 has an important converse. Again suppose ? : X —> Y is the
Serre fibration with which this topic began. Let my : (AVy,d) -=* Apl(Y) be
a Sullivan model, but now suppose given
• a relative Sullivan algebra (AVy,d) —> (AVy <g> AW,d).
• a cochain algebra morphism ? : (AVy <g> AW,d) —> Apl(X) that restricts
to ApLip)my in (AVy,d).
Then, as above, ApLU)n factors over ? · id to yield ? : (AW, d) —> Apl(F).
As in Theorem 15.3 we suppose now that Y is simply connected and that one
of H,(F;k),H*(Y;R) has finite type.
Proposition 15.6 If ? is a quasi-isomorphism then so is n, i.e.,
? : (AVy ® AW,d) —> APL(X)
is a Sullivan model for X.
proof: In the proof of Proposition 15.5 we showed that (AVy <g> AW, d) was a
Sullivan algebra. Thus it suffices to show that ? is a quasi-isomorphism.
Let m : (AVy <g> AV,d) -=» APL(X) be the Sullivan model of diagram A5.4).
Use Proposition 14.6 to construct a morphism of cochain algebras ? : (AVy ®
AW,d) —> (AVy ® AV,d) such that
? = id in AVy and ??? ~ ? rel (AVy,d) .
Now apply k ®AVy - to obtain a morphism ? : (AW,d) —> (AV,d) such
that ??? ~ n. Thus ?(??)?(?) = H(n). Since H(m) is an isomorphism
(Proposition 15.5) so is ?(?). Put I(k) = (A^kVy ® AW,d) and J(k) =
(A^Vy <8>AV,d). Then ? restricts to maps I(k) —» J(k). The induced maps
I(k)/I(k + 1) —> J(k)/J(k + 1) have the form
id®Tp: (AkVy,d0)®(AW,d) —> (A*Vy,d0) ® (AV,d),
200 15 Fibrations, homotopy groups and Lie group actions
where do '¦ AVy —> AVy is the 'linear part' of d. Thus these maps are all
quasi-isomorphisms.
An obvious induction on k now shows that ? induces quasi-isomorphisms
0(k) : (AVY ® AW,d)/I(k) -=» (AVY ® AV,d)/J(k)
for k > 1. Since Vy = {VY}i:>1 by the definition of a Sullivan algebra, I(k) and
J(k) are concentrated in degrees > k. Thus we may identify ??(?) = Hl(9(k))
for i < k, and so ? is a quasi-isomorphism too. Since ??? ~ n, so is n. ?
(b) Loops on spheres, Eilenberg-MacLane spaces and spherical fibra-
tions.
We apply the results of §15(a) to compute explicit Sullivan models in some
important, but elementary, examples.
Example 1 The model of the loop space QSk , k > 2.
Let ? : PSk —> Sk be the path space fibration, with fibre QSk. Ifk is odd the
minimal Sullivan model for Sk has the form ??$ : (A(e),0) -^ ^^E^). Define
m : (A(e,ti),du = e) —> APL{PSk)
by me = ApL(p)mse and mu = ?, where ? is any cochain satisfying d$ =
-^pl(p)ttis&· (Since PSk is contractible any cocycle of positive degree is a
coboundary.) By inspection, m is a quasi-isomorphism. Hence it follows from
Proposition 15.5 that m factors to yield a minimal Sullivan model
Thus H'(QSk; Jk) is the polynomial algebra on a class [u] of even degree k — 1.
// k is even then the minimal Sullivan model for Sk has the form m$ :
(A(e,e'),de' = e2) —> ApL(Sk). In this case ApL{p)ms extends to a quasi-
isomorphism
m : (A(e,e,u,u'), du = e, du' - e' - eu) —» APL(PSk).
Thus a minimal Sullivan model for ClSk is given by
m : (?(??,??'),?) -=> -4??(?5?).
In particular H'(USk;]k) is the tensor product of the exterior algebra on the
class [u] and the polynomial algebra on the class [u1]. Here deg[u] = k - 1 and
deg[u'] = 2k - 2.
Note that whether k is even or odd the Hubert series (§3(e)) of H*(QSk;Jk)
is given by
oo
„ ' 1 — Zk~1 '
Sullivan Models 2°1
Example 2 The model of an Eilenberg-MacLane space .
Let X be an Eilenberg-MacLane space of type (?, ?), ? > 1. Thus (cf. §4(f))
Wi(X) = 0 for i ? ? and there is a specified isomorphism ??(?) = ?. Assume ?
is abelian (this is automatic for ? > 1) and set Vn = ????(?, k). The Hurewicz
theorem 4.19 asserts that Hi(X;Z) = 0, 1 < i < ? and that the Hurewicz
map is a natural isomorphism ? —> Hn(X; Z). This extends to an isomorphism
? ®z k -=» Hn{X;Jk), which dualizes to an isomorphism Vn A- H"(X; k).
Now suppose ? ®% k is finite dimensional. We shall show that the minimal
Sullivan model of X is given by
m: (AVn,0) -=> APL(X)
where m induces the isomorphism Vn = Hn(X\k) above. This is clearly equiv-
alent to the classical assertion: H*(X;k) is the exterior algebra on Hn{X;k) if
? is odd and is the polynomial algebra on Hn(X;k) if ? is even.
The proof is by induction on n, beginning with ? = 1. Let a\,...,ar G ??
represent a basis of ? ®z k. These elements define a homomorphism a : ? ?
• · · ? ? —> ?. By Propositions 4.20 and 4.21 there is a continuous map / :
K{Zr, 2) —> ?(?,2) such that ??2(/) = a. Hence ?»(/) ®21· is an isomorphism,
and so 7?*(/) ®zQ is an isomorphism. Now apply Theorem 8-6 to conclude that
-ff*(H/;Q) is an isomorphism, whence also H*(uf;k) is an isomorphism.
But QK(n,2) is an Eilenberg-MacLane space of type (?, 1) and ??"(??,2)
is an Eilenberg-MacLane space of type (Zr, 1). Thus if ?' is any Eilenberg-
MacLane space of type (?, 1), X has the weak homotopy type of ??'(?,2),
by Proposition 4.21. Similarly S1 ? · · · ? 51 has the weak homotopy type of
QK(Zr,2). It follows that H*(X;k) ? H'iS1 ? · · · ? S1; A); i.e. is the exterior
algebra on r generators in degree one.
Now assume ? > 2 and that our result is established for ? — I. Let A' be an
Eilenberg-MacLane space of type (?, ?) with ? <g>z k finite dimensional. Then,
as above, UX is an Eilenberg-MacLane space of type (?, ? - 1). By induction
UX has a Sullivan model of the form (At/",!)) with Un~l = HomzGr,$:). In
particular, H,(ClX;k) has finite type.
Let (AW, d) be a minimal Sullivan model for X. Since H,(CtX;k) has finite
type we may apply Proposition 15.6 to obtain a quasi-isomorphism of the form
(AW ® Wn-\d) A APL(PX).
Moreover, since Hi(X;Z) = 0, 1 < i < n, it follows that ?^?-,?) = 0, 1 <
i < n. This implies (Proposition 12.2) that Wi = 0, 1 < k < n. By minimality,
d = Qin Wn.
On the other hand, the quasi-isomorphism just above shows that
H(AW^AUn-1,d) = k. Thus (AW ® AUn~\d) is a contractible Sullivan
algebra. It follows that d : Un~l A W and so W - Wn. D
Example 3 The rational homotopy type of K(Z,n).
202 15 Fibrations, homotopy groups and Lie group actions
Let K{Z,n) denote an Eilenberg-MacLane space of type (Z,n), and let an :
Sn —i K(Z,n) represent a generator of ?? (?(?, ?)) = ?. Then by the Hurewicz
theorem 4.19, Hn{an;Z) : Hn(Sn;Z) A Hn{K(ix,n);Z). Hence Hn(an;Q)
is also an isomorphism. Moreover, for ? > 2, ??? : USn —> CiK{Z,n) =
K{Z,n— 1) and ??_?(???) is an isomorphism, by the long exact homotopy
sequence applied to the path space fibrations. Hence, as above, Hn~1(Clan;Q)
is an isomorphism.
The computations of Examples 1 and 2 above now show that H* (a2n+i;Q)
and H* (Qa2n+i;Q) are isomorphisms, and so the Whitehead-Serre theorem 8.6
asserts that:
a2n+i : S2n+1 —» K(Z,2n+l) and ??2?+1 : ?52?+1 —> K(Z,2n)
are rational homotopy equivalences.
The reader is cautioned, however, that these maps are far from 'integral' homo-
topy equivalences. This illustrates the difference in complexity between integral
and rational homotopy theory. ?
Example 4 Spherical fibrations.
A spherical fibration is a fibration ? : X -> Y whose fibre has the homotopy
type of a sphere Sk. Suppose given such a fibration with simply connected base
Y.
If k is odd then the minimal model of Sk has the form (A(e),0). Hence we
can apply Theorem 15.3 to obtain a model for ? of the form
(Apl(Y) ® A(e),d) A APL(X), de = ? e APL(Y).
If k is even then the model of Sk has the form (A(e,e'),de' =¦ e2). Thus ?
has a model of the form (Apl(Y) <g> A(e.e'),d) with de G ???{?) and de1 =
e2 + ? <8> e 4- b for some elements o,6 € Apl(Y). The condition d2e' = 0 now
implies that 2de = -da. Replace e by e + ^a to obtain a model in which de = 0
and de' = e2 + z, some ? € Apl{Y)· In summary, ^.^^(p) has a model of the
form
(APL(Y)®A(e,e'),d) ^ APL(X), * = °
de' = e2 + ?, ? G ^??(?).
Note that in both cases ? is a cocycle in Apl (V) whose cohomology class [z] is
determined by the fibration. In particular this class is zero if and only if Apl (X)
is weakly equivalent to Apl(Y ? Sk). However in the case of even spheres an easy
calculation shows that H*(X;&) S H*(Y;k) ® H'(Sk) as H*(Y;k)-modules.
Finally suppose the spherical fibration arises as the unit sphere bundle of a
vector bundle ? : ? —> Y of rank k + 1. (For facts about vector bundles and
characteristic classes the reader is referred to [128] and [69] [70].) If k is odd the
class [2] is the Euler class of ?, essentially by definition.
If k is even then [2] = \p2kfe), where ?2?;(?) denotes the 2&th-Pontrjagin
class of ?. In fact, we can use ? : X -> Y to pull the vector bundle ? back to
Sullivan Models 203
a vector bundle ?? over ?, and ?? is the direct sum of a trivial line bundle
and a vector bundle ? of rank k. Let ? denote the Euler class of 77. Then
?2 = p2k(v) = H*(p)p-2h{0· Moreover, if 5* is the fibre of ? : Ar -» Y at y, then
77 restricts to the tangent bundle of S?. Hence, by a result of Hopf, (?, [Sy]) = 2.
On the other hand, it follows directly from the model that H*(p)[z] = [e]2
with ([e],[S?]) = 1, and that this condition determines [z] uniquely. Hence
Example 5 Complex projective spaces.
The inclusions S1 C S2 C · · · C Sr C · · · define a CW complex S°° = \JSr,
r
and it follows from the Cellular approximation theorem 1.2 that 7rrE°°) =
??E?+1) = 0, r > 0. Regard 52n+1 as the unit sphere in Cn. Complex multi-
plication defines a free action of S1 on each 52n+1 with orbit space the complex
projective space CPn. Similarly S1 acts on S°° with orbit space CP°°; and the
51-bundle 52n+1 —> <CPn is the restriction of the S^bundle S°° —> CP°°.
From the long exact homotopy sequence deduce that CP°° ~ K(Z,2) and
hence that i/*(CP°°;Q) = Au, with degu - 2 (Example 2, above). Similarly,
7r«(CPn) <8> Q = Qu © Qx with degu = 2 and degx = 2n + 1. Since CPn is a
2n-dimensional CW complex it has no cohomology in degree 2n + 2. Thus· its
minimal Sullivan model must have the form A.(u,x;dx = un+l). In particular,
CPn is formal with cohomology algebra Au/un+1. ?
(c) Pullbacks and maps of fibrations.
Suppose given any commutative square of continuous maps
? —s— x
?-?-y
in which p and q are Serre fibrations, ? and X are path connected and ^4 and
Y are simply ?onnected. Choose basepoints ao and yo so that /(ao) = yo and
denote by g : F —> F the restriction of g to a map from the fibre of q at ao to
the fibre of ? at yo·· Finally, assume that H,(—\ h) has finite type for one of F,
A and for one of F, Y.
Choose Sullivan models ??? : (AVy,d) —> Apl(Y) and nA : (AWA,d) -=>
APL(A), and let
?: (AVY,d) —? (AWA,d)
be a Sullivan representative for /; i.e., ??? ~ APL(f)mY. The Sullivan models
extend (A5.4) and Proposition 15.5) to commutative diagrams
204
15 Fibrations, homotopy groups and Lie group actions
APL(Y)
APL(X)
APL(F)
and
(.\Vy,d) —- (AVY®AV,d) —^ (AV,d)
ApL(A) -^^l APL(Z) - APL(F)
A5.7)
(AWA,d) — (AWA®AW,d) —* (AW,d)
in which all the vertical arrows are Sullivan models.
Suppose first that we have been able to choose ? so that
??? = ApL(f)my .
Then the pushout construction of §14(a) yields the morphism
? = ApL{q)nA-APL{g)m : (AWA,d) ®(Aiv,<0 (AVV ® AV'.d) —> APL(Z) .
This may be written as ? : (AWA <8> AV,d) —> APl(Z), and fits in the commu-
tative diagram
Apl(A)
Apl(Z)
Apl(F)
{AW? ® ??', d)
{AV,d)
Thus we may apply Proposition 15.6 to deduce
Proposition 15.8 If H*(g) : H*(F;k) —» H*(F;lc) is an isomorphism (in
particular if ? —> A is the pullback of X —> Y), then ? is a Sullivan model for
?. ?
Now consider the more general situation where ??? ~ Api(f)my. Here we
shall extend ? to a commutative diagram
(AVY,d) (AW®AV,<
?-id
(AV,d)
A5.9)
(AWAid) —- {AWA®AW,d) —^ (AW,d)
Sullivan Models 205
in which ? and ? are Sullivan representatives for g and for g; i.e. ?? ~ ApL(g)m
and ?~? ~ ^4pl(9)t?x.
Denote by ??,?? : — ® A(t,dt) —> — the morphisms (id ¦ Eq) : ? 4 0 and
(id · ??) : ? h-> 1. Let
be the fibre product with respect to the morphisms
ApL(Z) ® A(i,di) -?2» APL(Z) A- (ATF.4 ® AIF,d)
and let 0L and 0fi denote the projections of the fibre product on the left and right
factors. Now ?? is surjective and ker ?? = keroo. Hence H(kerQR) = 0 and gR
is a quasi-isomorphism. It follows that sqql = ??? is a quasi-isomorphism and
therefore so is ql .
Let ? : (AV'y,d) —> ApL(A)<8>A(t, dt) beahomotopy from ??? to ApL(f)my.
Then we have the commutative diagram
(AVy,d) — PL q———>- [Apl(Z) ® A(i, dt)] x.iPL(z) (AI-V.4 ® AW, d
s,eL A5.10)
(AVY ® AV, d) — ¦
Since ^L is a quasi-isomorphism so is ?^?1. Moreover, if ? G Apl(Z) then
?oB ® t) = 0 and so (z ® i, 0) is an element in the fibre product. Now S\QL{z ®
*>0) = E\(z ® i) = z, which shows that ?ie>l is surjective. By the Lifting lemma
14.4 there is a morphism
? = (?,?) : (AVy ® AV,d) —»· [.4PL(Z) ® A(i,di)] x^pl(z) (ATV4 ® ??-'.d)
extending (??/,(?)?,-0) and such that ?!^Lr = .4p?,(g)m.
In particular ??? = ?? and ?? ? = ??^? = ApL(g)m. Thus ? is a homotopy
from ?? to ApL(g)m and <^ : (AVy ® AV, d) —>· (?1?'".4 ® ???, d) is a Sullivan
representative for g. Moreover ? extends ? and so the left hand square of A5.9)
commutes.
Finally, set
? = &®??: (AV, d) —> (AW, d).
By construction, the right hand square of A5.9) commutes. Moreover, just as
m and ? factor to give m and ? so ? factors to define a morphism
? : (AV,d) —)¦ APL(F) © A(t,dt)
such that ??? = nTp and ??? = Api(g)fn. Thus nTp ~ .4pL(^)m and Tp is a
Sullivan representative for g. This completes the construction of A5.8).
206 15 Fibrations, homotopy groups and Lie group actions
Remark As noted in §15(a), the models in A5.7) may be chosen so that
{AVY,d) —> (AVY <g> AV,d) and (AWA,d) —> {AWA ® AW,d) are minimal
relative Sullivan algebras. In this case (id ·?) is a quasi-isomorphism between
minimal relative Sullivan algebras. Thus Theorem 14.11 asserts that
id -? : (AWA ? AV, d) A (hWA ® AW, d)
is an isomorphism of cochain algebras.
Example 1 The free loop space Xs1.
Let Ar be a simply connected topological space with rational homology of finite
type. The free loop space, Xs , is the topological space of all continuous maps
S1 —> X. We may identify these as the continuous maps f : I —> X such that
/@) = /(I) and this defines an inclusion i : Xs —> X1. Moreover
xs' { . ??
P(g) = 9@),
?(?) = (?,?)
? —^ ? ? ?
is a pullback diagram of fibrations. Finally, the constant map I —> pt defines a
homotopy equivalence X1 <— Xpt = X that converts the diagonal ? to q.
We may now apply the results above to compute a Sullivan model for X5 as
follows. Let (AV, d) be a minimal Sullivan model for X. Then multiplication
? : (AV, d) ® (AV, d) —> (AV, d)
is a Sullivan representative for ? as follows from Example 2, §12(a). Convert
this to a relative Sullivan algebra (AV ® AV ® AW, d) -=> (AV, d) and then by
Proposition 15.8, (AV,d) ®??®?? (AV ® AV ® AW,d) = (AV <g> KW,d) is a
Sullivan model for Xs .
In this case we can carry out these computations explicitly. Define V by
Vk = Vk+1 and consider the acyclic Sullivan algebra (E(V),d) = AV ® AdV
constructed in §12(b). In (AV, d) ® (E(V),d) define derivations s of degree -1
and ? of degree zero by sv = v, sv = sdv = 0, and ? = sd + ds. (Here the
isomorphism V = Vk+l is denoted by ? —> ?.) Then an isomorphism
? : AV ® AV ® AV A (AV, d) ® (E(V),d)
oo
is given by ?(? ® 1 <g> 1) = ?, ?A ® ? ® 1) = ? ^ and ?A ® 1 ® ?) = ?.
? " _
Now ? is a derivation satisfying ?? = ?? in AV ® E(V). It follows that
oo n
e& = ? 7?" is an automorphism of this graded differential algebra. Hence ?(\®
n=0
dv ® 1) = ??A ® ? ® 1). This implies that the inclusion,
? : (KV, d) ® (AV, d) —> (AV ® AV ® AV, d)
Sullivan Models 207
is a relative Sullivan algebra. The quasi-isomorphism (AV ® AV ® AV, d) ^>
(AV,d) ® (E(V),d) -=» (AV, d) converts ? into multiplication in AV, and so ?
is a Sullivan model for the multiplication morphism. Thus, as described above,
(AV ® AV, d) = {AV, d) ®??®\? (? V ® AV ® AV, d)
is a Sullivan model for Xs .
It remains to compute d. Observe that s = 0 in V and dV and hence that
s2 = 0 since s'2 is a derivation. It follows that
= v + dv + ^2
n=l
and hence that s' = ?~??? is the derivation in AV ® AF ® AV given by
?'(?® 1® 1) = ?'A®?® 1) = 1® 1®? and s'(
Thus in AV ® AV ® AV,
(
v + ?^
n=l
Finally, let s" be the derivation in AV ® AV given by s"v = ? and s"v = 0.
Then an immediate computation from this formula gives the differential d in
AV ® A V as:
dv = dv and dv = —s"dv .
This defines the Sullivan model for Xs explicitly in terms of (AV, d). ?
208 15 Fibrations, homotopy groups and Lie group actions
(d) Homotopy groups.
Suppose / : X —> Y is a continuous map between simply connected spaces,
and let
mx : {AVx,d) —> APL(X) and mY : {AVy,d) —> APL(Y)
be minimal Sullivan models. Let </?/ : (AVY,d) —> (AVx,d) be a Sullivan
representative for /; i.e., ????/ ~ ???(/)???. Its linear part,
QU) : VY -»¦ Fx
is independent of the choice of </?/ (§13(c)).
In §13(c) we introduced the bilinear map
defined by Q(a)v = (?; a)e, where a: Sk —> X represents a and (A(e,... ), d) is
the minimal model of Sk. This determines the linear map
ux :VX —>Homz (?*(*),*)
given by (uxv)(a) = (v;a). Given / : X —> Y, we have (Q(f)v;a) =
(?;?*(/)?), ? ? Vy, a G ?*(?). It follows that ?? is a natural transforma-
tion:
vx ? Q(f) = Homz (?.(/), ?) ? i/y.
The minimal Sullivan models approach to rational homotopy theory is suc-
cessful because ?? identifies the generating space of the model with the dual of
the homotopy groups of the space:
Theorem 15.11 Suppose X is simply connected and H»{X; h) has finite type.
Then the bilinear map ?? ? ?*(?) —> Jk is non-degenerate. Equivalently,
is an isomorphism.
Remark 1 It follows from Theorem 15.11 that if H*(X;<QI) has finite type so
does 7T,(X)®Q, since in this case the Sullivan model has finite type. Conversely,
if ?» (?') ®Q has finite type then the procedure for constructing a rational cellular
model Xq (§9) shows that #«(Ar;<Q>) has finite type. Indeed if we know by
induction that the r-skeleton of Xq is finite we can conclude that it has rational
homology (and hence rational homotopy) of finite type. Thus the (r+l)-skeleton
is constructed by adjoining finitely many rational cells.
Remark 2 Theorem 14.11 asserts that a morphism ? between minimal mod-
els is an isomorphism if and only if it is a quasi-isomorphism. Since ? is an
isomorphism if and only if its linear part Q((p) is an isomorphism we recover the
Sullivan Models 209
-rational Whitehead-Serre theorem for continuous maps / : A' —> Y; ??,(/) ® Q
is an isomorphism if and only if H*(f;Q) is an isomorphism.
proof of Theorem 15.11: Fix k > 2.
To show that ?/? : V? -=> Homz G?*(?"), Jfc) we let r be the least integer such
that ??(?) ? ?, and argue by induction on k — r.
If k = r then the Hurewicz homomorphism is an isomorphism ^r(A') —>
Hr{X\1) (Theorem 4.19). On the other hand, since H'l{X;k) = 0 for 1 <
i < r — 1 the minimal model for X satisfies Vx = 0, 1 < i < r — 1 (Proposi-
tion 12.2). Thus H(mx) : V{ A Hr(X;]k). It is immediate from the defini-
tion that these isomorphisms identify ?? with the isomorphism Hr(X;]k) —>
Homz (HT(X; Z), Ik). Thus when k = r, ? ? is an isomorphism.
Suppose now that k > r. Observe first that if f : X —> Y is a weak homotopy
equivalence then H*(f; Jk) is an isomorphism. It follows that a Sullivan represen-
tative ?/ is a quasi-isomorphism and hence an isomorphism (Theorem 14.11).
Thus Q(f), as well as ?«(/), are isomorphisms. Thus by naturality, we may
replace A" by any space of the same weak homotopy type.
In particular, we may suppose A" is a CW complex. Let ? be an Eilenberg-
MacLane space of type (nr(X),r). Choose a continuous map g : X —> ? such
that nr(g) = identity (Proposition 4.21). Factor g (as in §2(c)) in the form
? ?? ? ?
with ? a homotopy equivalence and ? a fibration. Again, use naturality to replace
X by ?" ?? ??. Thus we may assume there is a fibration
? : X —> ?
such that ?r{p) is an isomorphism.
In Example 2 of §15(b) we showed that the minimal model of ? has the
form (AFr,0) -=> APLiK). In particular, H*iK;Jk) has finite type and so
Theorem 15.3 and its consequences apply to the fibration p.
Now let j : F —> X be the inclusion of the fibre of p. By the long exact
homotopy sequence (Proposition 2.2), 7rr(F) = 0.
Because itiiF) = 0, 1 < i < r, a minimal model of F has the form mp '¦
(AV>,d) A APL{F) with VlF = 0, 1 < i < r (Proposition 12.2). Thus dia-
210 15 Fibrations, homotopy groups and Lie group actions
gram A5.4) for ? becomes
APL{K) ApL(p). ApL(X) AplU)> ApL(F)
{AVr®AVF,d) —r
L ?-id
Since Vp is concentrated in degrees > r the Sullivan algebra (AVr ® AVp,d) is
necessarily minimal. Lift ??? to a quasi-isomorphism ? : (AVx,d) -=> (AV ®
AVp,d) such that ??? ~ ??? and conclude from Theorem 14.11 that ? is an
isomorphism. Since H*(X; Ik) has finite type so does AVx (Proposition 12.2(iii)).
Hence so does AVp and, a fortiori, H*(F;lk).
Finally ???(? ¦ ??)? = ApLU)mip ~ ApL(j)mx. This identifies (? · ??)? as
a Sullivan representative for j. In particular, Qk(j) is the isomorphism Qk(e ¦
id)Qk((p). The long exact homotopy sequence for ? shows that nk{j) is also an
isomorphism. Thus ?? and vp are identified in degree k by naturality, and ??
is an isomorphism in degree k. D
Recall (§13(c)) that the projection A+Vx —> Vx induces a linear map ? :
H+(AVx) —> Vx. From the commutative diagram at the end of §13(c) we
deduce the
Corollary The isomorphisms ?? : Vx —> Homz (n*(X),k) and ?(???) :
? (AVx) ^» H*(X;h) identify ? with the dual of the Hurewicz homomorphism.
a
Example 1 Rational homotopy groups of spheres.
Let i G 7fn(Sn) be the class represented by the identity map of Sn. Then
_ ( Q-i ,n = 2/c+l
| 0 , otherwise
and
{Q-i ,n = 2k
Q'[t,t]w ,n = 4k-l
0 , otherwise.
Indeed in the first case the minimal model is (A(e),0) and (e;i) = 1. In the
second the minimal model is (A(e,e'), de' — e2). Again (e;t) = 1 while Propo-
sition 13.16 gives (e';[t,i]w) = -(e2;i,i) = -2. Now apply Theorem 15.11
D
Example 2 The model (?(??,??,?), dx = eoe-i), k = Q.
In Example 2 of §13(e) we considered the space X = (S3 V S3) U/?? U
D\) where the two 8-cells were attached respectively by [a0, [ao,ai]w\w and
Sullivan Models 211
[??, [ai,ao]w]vj/·· The minimal Sullivan model of ?' was shown to have the form
(AV,d) = (A(eo,ei,x,w,wo,---),d), where dx = eoei, dw = eoe\x and the Wi
have higher degree.
Thus a linear function V10 —> Q is defined by w h-> 1. By Theorem 15.11
there is a continuous map b : S10 —> X such that Q(b)w — Ae, some non-zero
? G Q. Put
Y = X Uf, Dn = (S3 V S3) U/ (D% II Df) u6 D11.
According to §13(d) the cochain algebra (AV ? ku,d?) is a commutative
model for Y, where ? = [b] G ????(?). A straightforward calculation shows that
a quasi-isomorphism
(AV ©Jku,d?) A (A(eo,ei,x),d)
is given byuH —A~1eoeix, m 4 0, «ij 4 0, i > 0. Thus (A(eo,e\,x),d) is the
minimal Sullivan model for Y. In particular,
,n = 3
Q ,n = 5
0 , otherwise.
Observe that the inclusion (A(eo,ei,x),d) —> (A(eo,ei,x,tu,... ),d) is a Sulli-
van representative for the inclusion i : X —> Y. This shows that Q(i) is injective
and so the dual map
?, (i) ® Q : ?» (X) ® Q —»¦ ?,(?) ® Q
is surjective.
Finally, we show that the homotopy fibre, F, of i has the same minimal
Sullivan model as a wedge of spheres, and compute its cohomology. Extend
(A(eo,ei,x),d) to the contractible Sullivan algebra (A(eo,ei,z,t;o,t>i,y),d) by
setting dvo = eo, dv\ = e\ and dy = ? + eoVi.
It follows from A4.6) that Q®A(eo,e,,x) (AV,d) is a Sullivan model for F. Form
the pushout
(A,d) = (A(eo,e1}x,vo,vi,y),d) ®A{eo,e],x) (AV,d)
as described in §14(a). On the one hand, (A,d) may be regarded as a relative
Sullivan algebra
(A(eo,ei,x,vo,vuy),d) —> (A(eo,...,y) ® A(w,wo,...),d).
Since ? : (A(eo,... ,y),d) —> Q is a quasi-isomorphism, so is ? ® — : (.4, d)
(A(u>, w0,... ), d). Thus (?, d) is a Sullivan model for F.
On the other hand, (.4, d) may be regarded as a relative Sullivan algebra
(AV,d) ^ (AV ® A(vo,vuy),d).
212 15 Fibrations, homotopy groups and Lie group actions
Now apply the quasi-isomorphism (AV,d) —> (A(eo,ei,x)/e§e\X,d) to obtain a
quasi-isomorphism
(A,d) ^ (C,d) = ([A(eo,ei,x)/eoeix]®A(wo,fi,i/),c0·
Since H (A(e0, · · ·, y), d) = Q, the short exact sequence
0 —> ((Qeoeix) ® A{vo.,Vi,y),O) —> (A(eo,ei,x,vo,vi,y),d) —> (C,d) —» 0
allows us to compute H(C), which is isomorphic to H*(F;Q). In particular, the
Hubert series of H*(F; <Q>) is given by
More precisely, the cocycles eixv^v^y ? C, k > 1, ? > 0, m > 0 represent
a basis of H+(C). Since these cocycles multiply each other to zero it follows
that H+(C) ¦ H+{C) — 0. Moreover these cocycles define a quasi-isomorphism
(H(C),0) —> (C,d) of cochain algebras. Hence (H(C),Q) is also a commutative
model for F, while by Proposition 3.4 it is also a commutative model for a wedge
of spheres. ?
Example 3 The Quillen plus construction .
Let X be a path connected topological space whose fundamental group, ?\ {?)
is finitely generated and such that every element in ix\ (X) is a product of com-
mutators. Then ??(?;?) = 0, by the Hurewicz theorem 4.19.
Adjoin to X finitely many two cells e?,...,e^ to kill a set of generators
of ?\(?). The Cellular approximation theorem 1.2 implies that ?\(?) —>
??\{? U LJe?) is surjective. Hence X U (Uef ) ^s simply connected. On the
i \ i /
other hand, since iii(X;Z) = 0, the long exact homology sequence for the pair
( X U '( U e? j ,X\ shows that there are homology classes ??,..., an ?
\ \ i / /
H2 I JU[Jef;ZJ that project to the classes [e?],..., [e^] in the relative ho-
mology.
Since XU ( |Je? j is simply connected the Hurewicz Theorem implies that the
ai can be represented by maps en : S2 —> X U ( \J e\ J. Attach three cells by
these maps to create the topological space Y = X U ? (J ef J U I (J e| J. This is
called the Quillen plus construction on X.
This construction has two important properties. Firstly Y is simply connected.
Secondly the inclusion X —> Y induces an isomorphism of homology, as follows
Sullivan Models 213
immediately from the long exact homology sequence for (Y,X). In particular in
the case X is an Eilenberg-MacLane space (and hence has no higher homotopy
groups) the higher homotopy groups of Y are invariants of the group ?\ (X); its
algebraic K-groups.
Now suppose the rational homology H*(X;Q) has finite type. Then we can
construct the minimal Sullivan model ??? : (AV,d) ^> Apl(X) and, as in
Proposition 12.2, V will be a graded vector space of finite type. However, unlike
the situation in Theorem 15.11, the graded vector space V may have little to do
with ?, (X).
On the other hand, the inclusion i : X —> Y induces a quasi-isomorphism
Apl(i) : Apl(Y) —> Apl(X)- Hence a Sullivan representative is an isomor-
phism of minimal models (Theorem 14.11), which exist by Theorem 14.12. Thus
Theorem 15.11 provides an isomorphism
?? :V -=>HomzGr.(y),Q) -
In particular the rational .fiT-groups of ?? (?) may be computed directly from
Apl{X)- When X is a smooth manifold we may also use Adr(X) as observed
in §12(e). ?
Example 4 Smooth manifolds and smooth maps.
Let X be a smooth manifold. In §11 we showed that Adr(X) is connected
by natural quasi-isomorphisms to Apl(X;R). Thus a minimal Sullivan model
(AV,d) ^> Adr(X) is a Sullivan model for X (over E). In particular, if X is
simply connected and H*(X; W) has finite type (e.g. if X is compact) then there
is an isomorphism
vx :l/-=>HomzGr.(X),R)
(Theorem 15.11). This shows that the real homotopy groups, as well as the
cohomology algebra, may be computed from Adr(X)-
Now let ? : X —> Y be a smooth map. In the same way, a Sullivan represen-
tative for Adr(<p) is a Sullivan representative for ?. Moreover, if Y is simply
connected and has real homology of finite type and if
m : (ADR(Y) ® AW,d) ^ ADR{X)
is a Sullivan model for Adr(<p), then we can apply Theorem 15.3 to identify
(AW, d) as a Sullivan model for the homotopy fibre of ?. (This, of course, need
not be a manifold !) D
(e) The long exact homotopy sequence .
Let ? : X —> y be a Serre fibration of path connected spaces, and with path
connected fibre j : F —> X. Assume that Y is simply connected and that all
spaces have homology #„(-; k) of finite type.
214 15 Fibrations, homotopy groups and Lie group actions
In §15(a) we constructed the diagram, labelled A5.4):
(AVY,d) —r~ (AVY®AV,d) -— {AV,d)
in which all three vertical morphisms are Sullivan models. Moreover we can (and
do here) take (AVy,d) and (AV,d) to be minimal. However, the central Sullivan
algebra, (A(VY @V),d) need not be minimal. Let do be the linear part of d: it
is the differential in V ? Vy defined by dx - dox G ?-2(V ? VY), ? ? Vo ? VY.
Then i and id -? restrict to a short exact sequence of cochain complexes,
0—> (VV,0) ? (VY©V,do) -^ (V,0) —»¦ 0. A5.12)
This construction is natural in the following sense. Given a map of Serre
fibrations, consider the Sullivan representatives constructed in A5.8). Their
linear parts define a morphism of the short exact sequences A5.12) and of the
corresponding long exact cohomology sequences.
Recall now from Theorem 14.9 (applied with ? = k) that {A{VY ® V),d) 9*
(AW, d) ® A(U ? dU) with (AW, d) a minimal Sullivan algebra and A(U ® dU)
contractible. This defines a quasi-isomorphism ? : (AW,d) -=> (A(VY ©V),d),
and ??? = m\ : (??^, d) -=* Api (X) is its minimal Sullivan model. Moreover
(Proposition 14.13), the linear part of ? induces an isomorphism W -^f H(VY ?
V,do). Use this to replace H(Vy ? V, do) by W in the long exact cohomology
sequence of A5.12), which then takes the form
Proposition 15.13 Suppose X and F are also simply connected. Then the
isomorphisms vY, vx and vF of Theorem 15.11 identify the long exact cohomol-
ogy sequence above, up to sign, with the dual of the long exact homotopy sequence
of the Serre fibration ? : X —>¦ Y.
proof: The linear maps VY ->¦ W and W -> V are identified with Homz (?*(?), k)
and Homz (?,(j),k), as follows easily from the definitions. To check that the
two connecting homomorphisms are identified we will verify that
(dov;a) = (-I)k+I(v;d*a), veVk, ae nk+1(Y).
Fix k and a, and let ? : Sk+1 —> Y represent a. Recall the path space
fibration ? : PSk+1 —> Sk+1. Since PSk+1 is contractible, const ~ ap. Cover
^;c hnmntnnv with a homotopy PSk+1 ? I —> X from the constant map to some
Sullivan Models 215
map b : PSk+1 —> X. Thus pb = ap and so b restricts to a map c : CiSk+1 —> F.
By naturality, it is sufficient to establish our formula for the fibration PSk+1 —>
Sk+1 and a = [idsk+i].
In this case, as we saw in Example 1, §12(a) (AVy,d) = (A(e),0) or (A(e, e'),
de' = e~), depending on whether k + 1 is odd or even. Moreover, as shown in
Example 1, §15(b), (AV,d) = (A(u),0) or (A(u,u'),O) and the differential in
(AV>- ® AV, d) is given by du = e and du' = e' — eu. In this case u is a basis for
Vk and dou = e, so that we are reduced to proving
Recall the continuous map ? : Sk ? I —> Sk+1 whose picture is given in
Example 5, §1. Regard ? as a based homotopy from the constant map to itself and
lift this through ? : PSk+1 —> Sk+1 to a based homotopy § : Sk ? I —> PSk+1
from the constant map to a map h : Sk —> QSk+1. Then [h] = d*[idSk+i].
Let zk € Ct(Sk;Z) be a cycle representing [Sk] — cf. §4(d). If ? is the
identity map of /, regarded as a singular 1-simplex then C*F)EZ(Zk ® ?) is
a cycle representing [5fc+1]. Set w = C»(e)EZ(zk ® t)· A quick computa-
tion shows that C*(p)w is the cycle representing [5fc+1] and that dw is a cy-
cle in C*(USk+1;Z) representing H*(h)[Sk]. Thus w projects to a relative
cycle w G Cm{PSk+1tUSk+1;Z). Moreover C7.(p) : Cm(PSk+ltUSk+1;Z) —>
C*(Sk+1,pt;Z) and we have, by construction that
H.(p)[w] = [Sk+1] and d.[w] = H*(h)[Sk];
here 9« also denotes the connecting homomorphism in homology for the pair
(psk+l,nsk+l).
On the other hand, the quasi-isomorphisms
? APL(PSk+1,nsk+1) «- APL(PSk+1) —- ApL(nsk+1) ·- ?
¦1=
(A+VV®AV,<i) -*¦ {AVy ®AV,d) (AV,d)
identify the connecting homomorphisms in the two long exact cohomology se-
quences. Moreover (cf. A0.13)) the upper long exact sequence is, up to sign,
dual to the long exact singular homology sequence. It follows that
(H(m)[e ® 1], [w]) = (H*(p)H(mY)[e], [w]) = (H(mY)[e],
Finally, since du = e ® 1,
(H{m)[e® 1],[UJ]) = (d*H(m)[u],[w}) = (-l)k+1(H(m)[u],Hk(h)[Sk]) .
_ But the Hurewicz homomorphism converts the duality between V and ?« to
the duality between cohomology and homology. Thus
(H(mY)[e],[Sk+1]) = (e;[ids^})
216 15 Fibrations, homotopy groups and Lie group actions
and
(H(m)[u], Hk(h)[Sk}) = (u; [A]> = (u; o,[id5*+.]>. ?
Return to the setup described at the start of this topic and recall that a linear
map C : H+{AV,d) -4 V is defined by ?[?] - ? G A^2F, ? a cocycle in A+F.
When F is simply connected this is dual to the Hurewicz homomorphism, hurp,
(§15(d)) and so cfoC is dual to hur? d„. We need this without the restriction that
F be simply connected:
Proposition 15.14 The linear maps doC and hurp d» are dual, up to sign, if
F is path connected and Y is simply connected.
proof: Let ? € (AF)* be a d-cocycle and let ? G ???+1 (Y) be represented by
? : Sk+1 —»?. We show that
(????·,?) = (-l)k+l(H(m){zlhurFd*a).
Use the pullback of the fibration via a, and naturality, to reduce to the case
Y - Sk+1 and a= idsfc+i. Then (AVY,d) = (A(e,...),cQ and dz = ???? = Xe,
for some ? G Jk.
Now the argument used in 15.13 for the path space fibration (with the last
paragraph suppressed) applies verbatim to give the proposition. D
(f) Principal bundles, homogeneous spaces and Lie group actions.
Let G be a path connected topological group with finite dimensional rational
homology. (This holds for all Lie groups.) As observed in Example 3, §12(a),
the minimal Sullivan model of G has the form
where Pg is a finite dimensional graded vector space concentrated in odd degrees.
Let xi,...,xr be a basis of Pg with degree Xi = 2lt + 1. Since G is weakly
equivalent to QBg (Proposition 2.10), there are maps f-L : S2li+1 —> G such that
{H(m.G)xj,H*(fi)[S2ei+1}} = 5i:j (apply Proposition 15.14). Thus we recover a
theorem of Serre: multiplication in G defines a rational homotopy equivalence
Now consider the universal principal bundle pg ¦' Eg —> Bq constructed in
§2(d). As described in §15(e) it determines a long exact sequence
connecting the generating spaces for minimal Sullivan models of Bg, Eg and G-
But H*{EG) = k and so W = 0. Thus d0 ¦" PG A VX+l. It follows that VBo is
Sullivan Models 217
finite dimensional and concentrated in even degrees. Thus AVbg is concentrated
in even degrees and so the differential must be zero. We have thus established
Proposition 15.15 The minimal Sullivan model for Bq has the form
where V?G = Pq+1 . In particular, H*(BG) is the finitely generated polynomial
algebra, AVbg- d
Since G is weakly equivalent to QBg, Bq is simply connected. Since (AV?G, 0)
is the Sullivan model of Bo-, it follows from Theorem 15.11 that V?G —
Homz GT«(i?G),¦&)· Thus tt«(Sg) ® Q is finite dimensional and concentrated
in even degrees and, for degree reasons, all the rational Whitehead products for
? g vanish.
Let yx,..., yr be the basis of Vbg corresponding to the basis x\,.. .,xr of Pg,
and define a contractible Sullivan algebra (AVbg <8> APg,cI) by setting dxi = yt
and dyi = 0. Since H*(Eg) = Ik, there is a commutative diagram of morphisms,
Apl(Bg) Apl{pg) ' APL{EG) - APL(G)
(AVBg,0) (AVBG®APG,d)
defined by setting ???{ = ?$, where ?$ G Apl{Eg) is anjr element satisfying
??? = ApL(pG)rriBGyi- Here m is a quasi-isomorphism by inspection and so
this is the special case of diagram A5.4) for the universal bundle. In particular
(Proposition 15.5), misa quasi-isomorphism. Thus we may take tug = ??.
Finally, suppose ? : X —> Y is any principal G-bundle over a simply connected
CW complex, Y. (The following could be extended to the non-simply connected
case.) This bundle is the pullback of the universal bundle pg ¦ Eg —> Bg over
a map / : Y —> Bg as shown in §2(e). The cohomology classes in Im H*(f) are
called the characteristic classes of the fibre bundle. Regard the basis yi, ¦ ¦ ¦ ,yr
of Vbg as cohomology classes of Bg, so that H*(f)y\,..., H*(f)yr are charac-
teristic classes.
Then a Sullivan representative
for / is characterized by the condition: ?/y-i is a cocycle in AVy representing
H*U) 1 < i < r. It defines the relative Sullivan algebra
(AVY,d) —> (AVY ® APG, d)., dxx = ?^ ;
218
15 Fibrations, homotopy groups and Lie group actions
which is just the pushout (AVy,d) ®(AvBG,d) (AV?G ®APG,d). Thus the Re-
marks in §15(c) give a commutative diagram
APL(Y) - Apl{p) - APL(X)
- APL{G)
(AVY,d)
(AVY®APG,d)
(???,?).
This identifies (AVy ® APG,d) as a Sullivan model for X.
Example 1 Homogeneous spaces.
Let ? C G be a closed subgroup of a connected Lie group G. Right multi-
plication by ? is an action on G and the projection ? : G —> G/K onto the
orbit space is the projection of a principal if-bundle [70]. The space (in fact, a
smooth manifold) G/K is called a homogeneous space.
Define a right action of ? on ?? x G by setting (?, ?) · b = (xb,b~xa). The
inclusion j : ? —> G defines an obvious continuous map E(j) : ?? —> Eg- The
formula (?, ?) h* (E(j)x) ¦ a defines a continuous map ?? xG —> Eg which
factors to yield a continuous map / : (?? x G)/K —> Eg- Thus we obtain a
commutative diagram
K x G)jK
BK
BG
in which q and B(j) are the maps of orbit spaces corresponding respectively to
the projection ?? x G —> ?? and to E(j). As in §2(e), g is the projection of a
principal G-bundle which the diagram exhibits as the pullback of the universal
bundle over B(j).
Suppose now that ? is connected too and use Sullivan models for ? ? and Bq
to identify
H*(B(j)) :
as a Sullivan representative for B(j). If yi,..., yr is a basis for V?G corresponding
to a basis xl,...,xr of Per then the discussion above exhibits
{AVBk ® APG, d),
dxi = H*
as a Sullivan model for (?? x G)jK.
On the other hand the projection ?? xG —> G induces (as in Proposition 2.9)
a weak homotopy equivalence q' : (?? x G)/K —> G/K. Thus G/K and
(V.v ? G)/K have isomorphic Sullivan models and we obtain
Sullivan Models 219
Proposition 15.16 The Sullivan algebra (AV?K ®APo,d) defined by dx{ =
H* (B(j)) y{ and d = 0 in Vbk is a Sullivan model for G/K. ?
Example 2 The Borel construction; application to free actions.
Let G be a connected Lie group. An action of G on a space X determines the
fibre bundle
q : XG —> BG
with fibre X, as described in §2(e). Now fibre bundles are Serre fibrations (Propo-
sition 2.6) and Bg is simply connected. Thus we may apply Proposition 15.5 to
this Serre fibration to obtain
APL(BG) Apl(9) > APL{XG) AplU) ' APL(X)
,0) - (AVBg®AVx,D) - (AVx,d)
in which ??? is a minimal Sullivan model for X. Thus the Borel construction,
Xg, has a Sullivan model of the form (AVBg ® ???,?) above. G
Proposition 15.17 If a compact connected Lie group acts smoothly and freely
on a manifold X then the Sullivan algebra (AVbg ® ???,?) is a Sullivan model
for the orbit space XjG. In particular, ?{ (AVBa ® AVX,D) = 0, i > dim X —
dimG.
proof: In this case the projection X —> X/G is the projection of a principal
G bundle [REF] and Proposition 2.9 provides a weak homotopy equivalence
q' : Xg —> X/G. This implies the first assertion. Since X/G is a manifold and
dim X/G = dim X — dim G, the second assertion follows. D
Example 3 Matrix Lie groups.
Compact matrix Lie groups
SO{n) C M(n; 1), SU(n) C M(n; C), Q(n) C M(n; M)
are defined as follows, where M, C, and H are the reals, complex numbers
and quaternions. Both C and H have a conjugations: a + ?i = a — ?i and
? + ?i + -yj + 5k = a - ?i - jj - Sk. Then
SO(n) = {A | A1 = A~X and detA = 1}
SU(n) = {???* = A~! and det.4 =
Q(n) =
220 15 Fibrations, homotopy groups and Lie group actions
The linear action of SO(n) in Rn restricts to a transitive action on 5n-1 which
identifies S0(n)/S0(n - 1) -=> S'1. Similar remarks apply in the other cases,
to give principal bundles:
S0(n - 1) —> SO(n) —> S71'1,
SU(n - 1) —> SU(n) —> S2" and
In particular 50B) ~ S1, SUB) s S3 and Q(l) = S7.
On the other hand, for any connected Lie group G with Sullivan model
(APg-.O) we have Pg — ^*{G) ® k as graded vector spaces (since G is weakly-
equivalent to QBg)· Thus the long exact homotopy sequences for the Serre fi-
brations give an easy inductive calculation of the Sullivan models of the classical
compact groups:
50B??. + 1)
S0Bn)
SU(n)
Q(n)
: ?(??,..
: ?(??,..
: A(x-2,..
: ?(??,..
¦,??)
.,??-?,? ,
¦,??)
¦,??)
???" ??. — Ai
UCg ? 2 **
? ? (? ?* · 4 7
degxi = 2i-
d.6?C ? ' — 4z
1.
1,
1.
1.
degx'n
Example 4 /4 fibre bundle with fibre S3 x SUC) that is not principal
The model for SUC) is A(x2,x3) with degx2 = 3 and degx3 = 5. Thus the
model for its classifying space is A(y-2,y3) with degt/2 = 4 and degj/3 = 6. These
elements are dual to maps Si -^» BSuC) and 56 —>¦ BSUCy
Consider the continuous map
/ : S3 ? S3 —> E3 ? 53)/E3 V S3) = S6 ? ?5?/C)
and use it to pull the universal bundle back to a principal 5f/C)-bundle ? :
X —»¦ S3 x S3. Let
g : ? -»¦ S3
be the composite of ? with projection on the left factor.
It is a relatively easy exercise to exhibit ? as a smooth fibre bundle, and a
theorem of Ehresmann then asserts that g is a smooth fibre bundle too. (It
is trivial that q is a Serre fibration.) The fibre of q is the compact Lie group
S3 x SUC). Now the discussion above gives a model for the principal bundle p,
from which we find that q is represented by
(Au,0) >¦ (A(u,V,X2,X3),d)
with degu = degu = 3; du = dv - dx2 = 0 and dx3 = uv. This is not the model
of a principal bundle and so q is not principal.
In Example 3, §14(d), we constructed a space Y = S3 V S3 U D8 U D8 U D10·
By inspection S3 x Y has the same Sullivan model as X. It is, in fact, easy to
see that X and 53 x Y have the same rational homotopy type. ?
Sullivan Models 221
Example 5 Non-existence of free actions.
Consider the manifold X of Example 4. We observed there that a certain
bundle q : X —>¦ S3 with fibre S3 x SUC) was not principal. Now we establish
a stronger assertion: X does not admit any free smooth S3 x SU(S) action.
In fact we show more: X has no free smooth S3 x S3 action. Indeed let
G = S3 x S3. For any G action the Borel construction has a Sullivan model of
the form
with dega,· = 4, and Dai = 0. For degree reasons Dx-$ = dx3 = uv and
Du,Dv G ?(??,??). Thus
0 = D2x3 = Du®v-Dv®u,
whence Du = Dv = 0. We also have Dxo, G ?(??, 02) and these calculations now
show that A(ai,ai)/(Dx2) is a subalgebra of H*(Xg)·
This subalgebra cannot be finite dimensional and hence (Proposition 15.17)
the action cannot be free. ?
Exercises
1 Compute a relative Sullivan model for:
a) the Hopf map 7 : Sr -> S4,
b) the composite S3 x S4 -> S3 ? S4 S S7 4 S4.
2 Consider a Serre fibration of path connected spaces F ? ? -> ?. Prove the
following assertions:
a) If Y = S3VS4VS5 and H*(F;Q) = ?(?1??2, ....,xk)/(xi,x'i ...,xl) with
each X{ of even degree then X ~q Y x F.
-.b) If if*(F; Q) = A(x)/(xfc) with x of even degree and k > 2 then the mor-
phism ii*(i) : H*(X;Q) -> H*(F;Q) is onto and if'(Ar;Q) is isomorphic as an
if*(F;Q)-module to the free module H*(Y;Q) ®H*(F;Q).
3. Consider for ? > 1, the group of unitary matrices: ^4 G U(n) if A = .d^1.
(A 0
Let ?/(&) c—y U(n + k) be the inclusion A ^ n r
a) Using the fibration U(n - l)->J7(n) -)· U(n)/U(n - 1) = 52"-1 prove
that f/(n) admits a minimal model of the form (?(?1,0:3, ...,a;2n-i),0) with deg
Zi = i. Compute the minimal model of the classifying space BU(n).
b) Using the fibration S2* -> f/(n + Jb)/J7(ife) -> J7(n + k)/U(k - 1) prove,
by induction on n, that H*(U(n + k)/U(k));Q) = A(x2fc+ljx2Jt+3,-,a:2n+2i-i).
4. Let F be the homotopy fiber of the inclusion of the ?-skeleton into the
classifying space X = BU(n). It is known that H*(F;Q) is the cohomology of the
"Lie algebra of formal vector fields on n-variables". Compute this cohomology.
222 15 Fibrations, homotopy groups and Lie group actions
5. Recall that for ? > 1, the nth-Postnikov approximation fn : X -» Xn of a
topological space X is defined by the following properties : ?^(/?) is an isomor-
phism for k < ? and nk(Xn) = 0 for i > ? + 1. Construct a relative minimal
model for fn. Deduce that a Sullivan minimal model of the homotopy fibre of fn
is quasi-isomorphic to the quotient differential graded algebra ifc<g>Ay<n (AV,d).
6. Let X be a 1-connected finite CW complex and ? > 1 and let ? be a
Sullivan representative of a continuous map / : K(Z, 2ri) —> X. Prove that ? is
homotopic to the trivial map.
16 The loop space homology algebra
In this section the ground ring is an arbitrary field ic of characteristic zero.
In this section we consider based topological spaces, (X,x0), and we shall
frequently assume that
X is simply connected and Ht(X;k) has finite type, A6.1)
even though a number of the results can, with additional work, be established
for a larger class of spaces. We shall also simplify notation and write
7?.??®* = 7?.(?')®?*.
As described in §2, composition of paths defines a continuous associative mul-
tiplication ? : ?? ? ?? —> ?? in the (Moore) loop space ?? and makes the
path space fibration PX —> X into an ??-fibration. The identity element of
?? is the constant path eo of length zero at Xo, and we shall always use eo as
the basepoint of ??.
The connecting homomorphism for the path space fibration is an isomorphism
0. :?.(?) -=>??._?(??),
since PX is contractible. In particular, ? ? (??) is abelian.
The geometric product ? : ?? ? ?? —> ?? makes C*(QX;Jt) into a chain
algebra via the Eilenberg-Zilber morphism EZ, as described in §8(a). The mul-
tiplication in the homology algebra, Hm(QX;Je), is given by
? · ? = ?*{?)?{??){?® ?), ?, ? G ?^??-k) ,
and the graded algebra H»(UX;Je) is called the loop space homology algebra of
X.
In this section we establish some strong structural results both for the coho-
mology algebra H*(QX;]k) and the homology algebra H»(flX; h) for any space
X satisfying A6.1). When X is a finite complex, however, its loop space ho-
mology has many more remarkable and beautiful properties. Developing these
is one of the main objectives of Part V of this text.
The structural results to be shown here are summarized by:
• H*(QX;Je) is a free graded commutative algebra.
• The Hurewicz homomorphism is an isomorphism
onto the primitive space for ??.
The graded algebra ?» (??;3?) can be computed explicitly from the graded
vector space ?, (X) <g> h and the Whitehead product map
w :G?.(?)®?)®(?.(?')®?) —> ?.(?) ® Jfc.
224 16 The loop space homology algebra
The first assertion is due (essentially) to Hopf and the last two to Cartan-
Serre-Milnor-Moore.
This section is organized into the following topics:
(a) The loop space homology algebra.
(b) The minimal Sullivan model of the path space fibration.
(c) The rational product decomposition of UX.
(d) The primitive subspace of H»(QX;]k).
(e) Whitehead products, commutators and the algebra structure of ?,(??; Jk).
(a) The loop space homology algebra.
Let (Y, y0) be a based path connected space. Recall (§3(b)) that the tensor
product of graded algebras A and ? is the graded algebra A <8> ? with (a <g>
b)(ai <8>&i) = (-l)de86de6aiua1 ®bh. Recall also (§3 (e)) that since A is a field,
homology commutes with tensor products.
Lemma 16.2
(i) If f : (X,xq) —> (Y,Vo) is continuous then Hr(uf;]k) is a morphism of
graded algebras.
(it) H(EZ) : ?.(??;]?)®?.(??;*) -=»¦ ?.(?? ? ??; A) is an isomorphism
of graded algebras.
proof: The first assertion follows from the naturality of EZ and the fact that
?/ is a morphism of topological monoids. The second assertion is a trivial
consequence of formula D.8) in §4(b). D
Next, recall from §4(b) that the topological diagonal Atop : Y -> ? ? ?,
y ¦->¦ (y^y)j induces the Alexander-Whitney diagonal
? = AW ? C.(Atop) : C*(Y; A) —* C.(K; A) ® C.(Y; A).
Since homology commutes with tensor products, ii»(A; A) is a linear map
H.(A;k) :Ht(Y;k) —>¦ H.(Y;
For simplicity we abbreviate #.(?; A) to ? (A).
In §4(b) we observed that (C*(Y;&),A) is a differential graded coalgebra,
co-augmented by any y G Y. Passing to homology, we see that H (A) makes
Ht(Y; A) into a graded coalgebra, co-augmented by any [y] ? H0(Y;Jk). If Y is
Sullivan Models 225
path connected these homology classes all coincide and we denote them by 1. In
this case we have for all ? G H+(Y;k) that
H(A)a - (a <g> 1 + 1 <g> a) G H+ (Y; k) ® H+ (Y; k) ,
as observed in §3(d). In particular, the element ? is primitive if
H(A)a = ? <8>1 + 1<8>?
and the primitive elements form a graded subspace Pt(Y;k) C H+(Y;k), the
primitive subspace of H*(Y: k).
Remark Note that the product (§5) in the cohomology algebra H*(Y;]k) is
dual to the comultiplication ? (A).
Lemma 16.3 If Y = UX thenH(A) : H.(ilX;k) —» H.(nX;k)®H.(nX;k)
is a morphism of graded algebras.
proof: Observe that ?? ? ?? is a topological monoid with component-wise
multiplication, and that ???? is a morphism of topological monoids. It follows
that Ht(Atop) : Ht(QX;k) —> Ht(UX x UX;k) is a morphism of graded
algebras. On the other hand H(AW) = H(EZ)~l as shown in Proposition 4.10.
Since H(EZ) is a morphism of graded algebras (Lemma 16.2) so is ? (AW).
Hence so is ? (A). ?
Example 1 The homology algebra H9(QS2k+l;k) , k > 1.
Let / : S2k —* QS2k+1 satisfy [/] = dt[idS2k+,}. Then the Hurewicz theo-
rem 4.19 asserts that H* (f)[S2k] is a non-zero homology class ? G H2k(^S2k+1 ; k)
The inclusion of ? extends to a unique morphism from the polynomial algebra
h[a}. We show this is an isomorphism:
In fact [S2k] is (trivially) primitive in Ht(S2k-]k) and so ? is primitive in
H*(QS2k+1;Jk). Since ? (A) is an algebra morphism, and since k is even,
H(A)an = (a <g> 1 + 1 <g> a)n = an <g> 1 + ???~? <g> ? + · · · + 1 <g> ?", ? > 1.
If ?" ? 0 then it follows that H(A)an ? 0 and so a" / 0. Hence our
morphism is injective. But in Example 1, §15(b) we computed the Hubert series
diH'(nS*k+1;k) to be (l - z2k)~l. Thus
A 2 2rk
= dim k[a]i.
0 , otherwise
It follows that the morphism above is an isomorphism. At the end of §16(d) we
establish the same result for Ht (QS2k ; Jfe). ?
226 16 The loop space homology algebra
(b) The minimal Sullivan model of the path space fibration .
Suppose (?,??) is a based topological space satisfying A6.1) and let ??? :
(AVx,d) —> Apl(X) be a minimal Sullivan model for X. Then the path space,
PX, has a Sullivan model of the form
m : (AVx ®AV,d) -=> ApL(PX).
It factors to give a minimal Sullivan model rn : (AV, d) —> Api(QX) for the loop
space CIX (the Corollary to Proposition 15.5 applied to the path space fibration
PX —y X). As in §15(e) recall that the linear part of d is the differential d0 in
Vx@V defined by requiring (d — dQ)x ? A-2(Vx © V). Because of the minimality
of (AVx,d) and (AV,d) we have d0 = 0 in Vx and d0 : V —» Vx.
Now observe that
d0 : V A VX.
Indeed, (AVx <g> AV, d) is the tensor product of a contractible algebra and a
minimal model for PX (Theorem 14.9). Because PX is contractible the minimal
model is trivial and (AVx <8> AV, d) itself is contractible. This implies H(Vx ®
V, d0) = 0 and hence that d0 : V A Vx.
Next, recall that Vx is a graded vector space of finite type (Proposition 12.2).
Hence so is H"(UX;Jc) = H(AV~d). Since UX is an ii-space it follows (Exam-
ple 3, §l2(a)) that the differential d is zero,
m: (AV,0) -=*???.(??).
Thus d : A(VX®V) —» A+V*- <g>AV. In particular, H*(QX; k) is the free graded
commutative algebra AV.
Moreover, if 2fa ,2k2,... and 2ix + 1,2?2 + 1,... are the degrees of a basis of
V then the Hubert series for H*(tlX;]k) has the form
Next observe that the action of UX on PX is a map of fibrations,
PX ? ?? 9—~ PX
X X
idx
where pL denotes projection on the left factor, PX. The tensor product of
Sullivan models is a Sullivan model for the topological product (Example 2,
§12(a)) and so
m-m: (AVX <g> AV,d) <g> (AV,0) -=> APL(PX x UX)
Sullivan Models 227
is a Sullivan model.
The restriction of g to the fibres at x0 is the multiplication ? : ?.? ? ?.? —y
??. Thus the construction of diagram A5.9) gives a commutative diagram
(AVx,d) (AVx®AV,d) (AV,0)
(AVx,d) —- {AVX <g> AV, d) ® (AV, 0) (AV, 0) <g> (AV, 0)
in which ? is a Sullivan representative for ?. In particular, (??·??)? ~ ???(?)??,
and so H(m) : AV -^ H*(QX; h) identifies ? with the dual of the multiplication
map for the graded algebra ?*(??;&). The fact that 1 6 ?0(??;&) is the
identity element translates to
^?-(?<8>1 + 1<8>?) G A+F<g>A+F, ? e A+V. A6.5)
Recall next (§13(e)) that the quadratic part of the differential d in AVx is the
derivation d\ determined by the two conditions
di-.Vx —> A2VX and d-dx:Vx—> A*ZVX.
In §13(e) we showed how to compute di from Whitehead products. Here we
show how to compute di using the map ?.
Let ? €V and, as in A6.5) above, write
?? = ? <g> 1 + ??, ® ?, + 1 <g> ?,
with ??,?, G A+V. Recall that ? : A+V —y V is the projection corresponding
to the decomposition A+V = F © ?-2 V and that d0 : V -^ Vx.
Proposition 16.6 The quadratic part of the differential in AVx is given by
dxdov = ?(-!)*«¦i(doC*i) ? (doCVi).
proof: Write dv = dov + ? u, ® ^ + ? + ? with u, € V*·, Uj € V, ? e ?
i
and ? € A^3(Vx ? F). Since d2v = 0 the component of cPu in A2(Vx ? V) is
zero, and this fact translates to
On the other hand, since ??? = (d <8> ??)??, the components of tpdv and
{d®id)ipv in Vx®\®V also coincide. Use the following facts to compute these
components:
228 16 The loop space homology algebra
• Im d C i\+Vx <8> AV · Im(^? - id ®?) C ?+ Vx <g> AV <g> AV
• ?= id in kVx · TpVi - 1 ® ?? 6 A+V ® AV.
This gives the equation Euj(8>li8>Vi = ?^???????&???, whence J](-l)desUiUiA
do^i = -?(-l)deg<i>i(doC$i) ? (doC*.·)· D
t
(c) The rational product decomposition of ??".
We turn the first part of the algebra above into geometry and provide a geo-
metric proof of A6.4) by identif3ring ??' as (rationally) a product of odd spheres
and loop spaces of odd spheres.
First we need to make some remarks about infinite products. Suppose
(Xa:*)acj is a family of based topological spaces. Let ?(??, ... ,ar) C Y\Xa
a
be the subspace of points (xa) such that xQ = * if ? ? ??7...,??; clearly
? (??,..., ??) — ? ?? ? · · · ? X<*r ¦ Let ? ?? be the union of the ?(??,..., ??)
?
as {??,..., ??} ranges over all finite subsets of J and give it the weak topology
determined by the X(cti, ¦ ¦ ¦ ,??). This space is called the weak product of the
(Xa,*)-
Any compact subspace of f[ Xa is contained in some X(a\,..., ??), so ]1 Xa
a a
is indeed a fc-space. Moreover, if each Xa is a CW complex with * 6 (Xa)o then
so is each X(a\,. ¦ ¦ ,??), and this makes f[ Xa into a based CW complex.
a
It also follows that
and so if each Xa is an Eilenberg-MacLane space K(Va;n) then Y[Xa is a
a
K(@Va,n). Finally, given a topological monoid G with unit element e and
a
continuous based maps fa : (Xa, *) —y (G, e) we define
(/?) : ? ?? -> G, (fa)(Xa) = T\UM ¦
a a
Now we return to the study of ??. First, suppose (X, *) is an arbitrary simply
connected based topological space. Every based map ? : Sk+1 —y X determines
two maps: ?? ·. USk+1 —* ?? and ? : Sk —> ??, where [?] = 3,[?] is the
image of [a] in ?.(??) under the connecting homomorphism for the path space
fibration. Moreover, if id : Sh+1 —» Sk+1 is the identity then a ~ uaold by the
naturality of <9». Let bt : S2?{+'2 —» X and a,· : S2k'+1 —* X represent a basis
of ?, (X) <g> Q. Multiplication of the b, and the ??^· defines a continuous map
/ = Fi) · (?^) : ?{52'·+1 x UjUS2^1 -> ??.
Sullivan Models 229
Proposition 16.7 Both ??,(/) <8> k and Ht(f;k) are isomorphisms.
proof: Since 3c is a field of characteristic zero it is sufficient to prove this for
Je = Q. Recall (Example 1, §15(d)) that 7r,(S2n+1) <8> Q = Q · [td52-+i]. It is
immediate from this and the construction that ?»(/) <8> Q is an isomorphism. If
??' is simply connected the Whitehead-Serre theorem 8.6 asserts that H*(f;Q)
is an isomorphism. To prove this when ??' is connected we may replace X by
any other space with the same weak homotopy type.
Let ? be an Eilenberg-MacLane space of type (?2(?"), 2), and choose a cellular
model X' for X (§l(a)). Choose a map ? : X' —> ? such that ?-2(?) = identity
(Proposition 4.20) and convert ? to a fibration (§2(c)). In this way we reduce to
the case that there is a fibration q : X —> ? whose fibre, F, is 2-connected.
Write the infinite product above as ? ? S} ) x ?, where ? is the weak product
\ i /
"of the odd spheres of higher dimension and the loop spaces ?52*?+1. Then
we may construct / so that it restricts to /i : ? —y ClF with ?»(/?) ® Q
also an isomorphism. By the Whitehead-Serre Theorem 8.6, i2,(/i;Q) is an
isomorphism.
On the other hand, Clq : ??' —> UK is an ?^-?^?????? (§2(b)). Let /2 be
the restriction of / to f] 5| and put g = {iiq)fc -YlSj —> ClK. Then define a
i i
map of ?^-?^^?????
f[S}xQF 5 *??
by setting h(x,y) = f(x) ¦ y. Since h ? (id x/i) = /, it is sufficient to prove that
H*(h;Q) is an isomorphism. This will follow from Theorem 8.5 if H*(g;Q) is an
~ / \ / ?
isomorphism. But we can write fl sl = ?' ??,? = UK ? ? ?,2 I. Hence
i V ? / \ i J
g ~ ?^?, where 7ro(<?i) = ^1E). In particular, ^2E1) ® Q is an isomorphism,
and so the Whitehead-Serre theorem asserts that Ht(Qgl;<Q) is an isomorphism
too. D
In summary: the map / is a rational homotopy equivalence. Thus loop spaces
have the rational homotopy type of a weak product of odd spheres and loop
spaces on odd spheres. Moreover Proposition 10.7 has the following
Corollary Let X be any simply connected topological space. Then there is a
rational homotopy equivalence of the form
? ?? -»¦ ??'
230 16 The loop space homology algebra
where ?,(Kn) ®Q is concentrated in degree n. ?
We now suppose that H*(X;Q) has finite type. Then (Remark 1 following
Theorem 15.11) ?*(?) ® Q has finite type and there are finitely many odd
spheres (or loop spaces on odd spheres) of any given dimension in the weak
product of Proposition 16.7. Thus a simple modification of the argument for
finite products (Example 2, §12(a)) shows that the minimal Sullivan model for
QX is the tensor product of the minimal Sullivan models for the S2fi+1 and
the QS2k} + 1. In particular the form of a minimal Sullivan model for ??' and
formula A6.4) could equally well have been deduced from Proposition 16.7.
Next, define homology classes /?,· and ay in H*(QX;k) by setting
?{ = Ht(bi)[S2ei+1] and ay = tf.(ay)[S2H
A homology class in H*(UX; ic) is called distinguished if it can be written in the
form
h < ¦¦¦ <ip,
ni,...,nq G N,
with ?, q > 0. (If ? = 0 there are no /Vs in the expression, and if q — 0 there are
no ay's. When ? = q = 0 there is a single class 7 = 1.)
Proposition 16.8 The distinguished homology classes ??? ?ip -?^1 ?"9
are a basis of the graded vector space Ht(QX;]k).
proof: Recall that oy ~_ (Qa.j)Jd, where id : S2ki —> CiS2hj+1 represents
d.[idsns+i]. Denote Ht(Id)[S2k^] simply by [5,-] G ?.(?52*?+1; Jfe). The classes
1, [Sj], [Sj]2, ¦.. are a basis for Ht(ilS-k:i+l ; h), as we showed in the Example at
the start of §16. Denote [52<i+1] simply by [Si], so that 1 and [5,] are a basis
for H»{S2ti+l;k). Then (Eilenberg-Zilber) the classes
are a basis for Hm ? s2Ii+1 x ? ??2*^1; ? . This basis is mapped by H.(f)
\i 3 J
onto the set of distinguished homology classes in H*(QX;lk). ?
(d) The primitive subspace of H*(QX;Jk).
Again suppose X is simply connected with rational homology of finite type.
Recall from §16(a) that H(m) : AV ? ?*(??;&), and that if [z],[w] G
H*(nX;k) and ? G H+(QX;k) then
([z)U[w],a) = {[z]®[w],H(A)a).
Thus ? is primitive if and only if {[z] U [w], a) = 0 for all [z], [w] G ?+(??; Se),
i.e., if and only if {H{fn)(A^2V),a) = 0. There follows
Sullivan Models 231
Lemma 16.9 A non-degenerate pairing between V and P*(QX:k) is given by
(v,a)>—> (?(??)?,?). ?
Next note that if / : Y —> ? is a continuous map between path connected
topological spaces, then #*(/) restricts to a map P*(Y;k) —> Pr(Z;k), be-
cause the Alexander-Whitney diagonal is natural. Moreover, [Sk] € Hk(Sk;]k)
is primitive because Hi(Sk;Jk) = 0, 1 < i < k — 1. Hence if a : Sk -> Y then
hurY([a\) = H*(a)[Sk] is a primitive element in H*(Y;k). Thus we may regard
the Hurewicz homomorphism as a linear map
7r.(y) —>P.(y;Jfc).
Recall that the topological space X we are considering is simply connected
and has homology of finite type (hypothesis A6.1)).
Theorem 16.10 (Cartan-Serre) The Hurewicz homomorphism extends to an
isomorphism
proof: We begin with three simple observations.
(i) hur : it* (S2e+1 ) <g> Jfe A P,(S2/+1; Jfc), i > 0.
Indeed, the Hurewicz theorem 4.19 asserts this is an isomorphism in degrees
< 2^+1. The homology of S2e+i vanishes in higher degrees D.14) as does
?»E2?+1) ® Jfc (Example 1, §15(d)).
(ii) hur : 7T*(OS2fc+1) ® Jfc ? ??(?52?+1; Jfc), fc > 1.
Since 7r*(OS2fc+1) ® A S ?*+?E2?:+1) ® h we conclude, as in (i) that this
graded vector space is concentrated in degree 2k. Thus the Hurewicz Theorem
4.19 asserts that hur : ?*(?52?'+1) ®?? ?2*(?52*+1;.?:). In the Example at
the start of §16 we saw that H*(QS2k+l;Ic) = k[a} with dega = 2k, and that
?(?)?? = ? [ ¦ ) a* ® an~l- This shows that an is not primitive for ? > 2,
i=o V z /
so that ?.(?52*+1;?) = ?2*(?52?+1; Jfc).
(Hi) If Y and ? are path connected topological spaces, then
?7??) = ??G)8?»(?) and P*(Y ? Z;k) = P*{Y;k) 0 P*{Z;k).
The first assertion is immediate from the definition of ?*. For the second, recall
that Eilenberg-Zilber induces an isomorphism H*(Y; k)®H*(Z; k) -=*¦ H*(Y ?
232 16 The loop space homology algebra
Z;k). It follows easily from formula D.9), §4(b) that this is an isomorphism of
graded coalgebras.
Let ? : H*(—;k) —> k be the augmentation induced by the constant map to
a point. Thus
(id -??) ® {?? · id) : [H.(Y; k) ® H.(Z;k)} ® [Hr(Y; k) ® H*(Z;k)}
—> H.(Y;k)®H.(Z;k).
But if 7 € Hr(Y;k) <S> Hr(Z;k) and if ?® denotes the comultiplication in the
tensor product coalgebra then clearly
(id -??) ® (?? ¦ id) ? ?® G) = y.
Hence if 7 is primitive then 7 € (if+(F;J;) ® 1) ? A ® ii+(Z;A)) and so 7 €
Now recall the continuous map
/ : ? S'2ei+1 ? ? fiS2fc'+1 —* ??
» j
of Proposition 16.7 and denote this, for simplicity, by / : Y —> (IX. The three
observations above imply that
hur :?.(?)® Jfe Apt(y;i).
Since ?*(/) ® A is an isomorphism and since the isomorphism H,(f;k) neces-
sarily restricts to an isomorphism P*(Y; k) -=+ ?*(??; k), the theorem follows.
(e) Whitehead products, commutators, and the algebra structure of
H.(flX;k).
Again suppose X is simply connected with rational homology of finite type.
The commutator of two elements ? and r in a graded algebra is the element
[?, r] defined by
[?,?] = ??- (-1)?^??^???.
Recall (§16(c)) that as a graded vector space, H*(QX;k) has a distinguished
basis consisting of the elements of the form
ii < ¦ ¦ ¦ < ip,
ni,... ,nq € ?.
Thus the algebra structure of ??(?.?; k) would be completely determined if we
knew the commutators
[?u?i·], [?uocj] and [uj.uj·.].
Sullivan Models 233
(Since deg?i is odd, ?f = \[?u?i].)
We shall express these simply and explicitly in terms of Whitehead products
(§13(e)), using the linear map ? = hur od* : ?*(A') —>¦ #*_?(??; Jfe).
Proposition 16.11 J/70 € iTk+i(X) and 71 € ??+?(?) then
proof: In Proposition 16.6 we showed that the quadratic part of the differential
in ??? was given by
di d0 ? = ?^(-1)?^^(?????) ? (doC*i), « € V,
i
where ^u = ? ® 1 + ???- ® ?? + 1 <g> ?. Since d0C is dual to ? (Proposition 15.14)
and since ? is dual to the multiplication in ?*(??; Je), we have
{?(??)?,[???,???}) = {?(??)?, (o
)?? ® ii(m)*i
^?··<#(??)?;, 07o)(ii(m)*<J
On the other hand, we may apply Proposition 13.16 where we calculated the
Whitehead product to obtain
(H(m)v; 0[7o,7iM = (-l)k+n+1 (dov; [7o,
The Proposition follows from these formulae, given the duality between V and
P»(nX;ic) established in Lemma 16.9. D
Finally, suppose given ?*(?) <8> h together with the Whitehead products. De-
fine a graded vector space ?? by setting (Lx)k = 7r^+1(A') ® k and denote by
234 16 The loop space homology algebra
sx € ?*(?) (g) k the element corresponding to ? € ??. In the tensor algebra
TLx let / be the (two sided) ideal generated by elements of the form
(-l)^***-1 ([sx,sy]w). A6.12)
Theorem 16.13 The Hurewicz homomorphism extends uniquely to an isomor-
phism of graded algebras
proof: First observe that an identification ?,? = ir*(iiX) ® Je is specified
by identifying ? with dtsx, ? € Lx. This identifies hurcix as a linear map
Lx —> H*(QX;]k), which then automatically extends to a morphism TLx —>
H*(QX;]k) of graded algebras. Proposition 16.11 asserts precisely that the el-
ements A6.12) are in the kernel of this morphism. Hence it factors over the
quotient TLx —> (TLx)/I to define a morphism of graded algebras
? : (TLx)/I —> H.(ClX]k).
We have to show that ? is an isomorphism.
As in §16(b) let bt : S2ei+2 —» X and a.j : S2k>+1 —» X represent a basis of
Tt*(X)®k. Put yi = s^] and Xj = s" [a,·]. Thus {yi,Xj} is a basis of Lx.
Recall that TSLX = Lx <g) · ¦ ¦ ® Lx (s factors). Let Fs C (TLX)/I be the
image of T~sLx; elements in Fs have filtration degree s. By the very definition of
7, if z,w,? Lx then zw - (-l)des-deswwz has filtration length 1. In particular
if ? has odd degree then z2 = \{zz + zz) has filtration degree 1.
An obvious induction on filtration degree now shows that every element in
(TLx)/1 is a linear combination of the elements
i\ < ¦ ¦ ¦ < ip
Vix yip' ?7? *J * ii < " " " < h
nt G N.
(Show that the subset with ? + ??^ < s spans Fs.) The morphism ? maps
these elements to the distinguished basis of ?*(??; &) established in Proposi-
tion 16.8. Hence the elements above must be a basis of (TLx)/I and ? must be
an isomorphism. D
Example 1 The homology algebra H,(USn+1;3z).
Let a: Sn —> QSn+1 represent d.[idSn+i], and let ? = hur[a] = H*(a)[Sn] ?
Hn(CtSn;h). We show that the inclusion of ? extends to an isomorphism
k[a] A H,(QSn+1 ; Jfe)
Sullivan Models 235
of graded algebras. If ? is odd this has already been done in the example at the
start of §16.
Suppose ? = 2k. Let ? = [ids2"] € n2k(S2k). Then n*(S2k) <g> Jk is two
dimensional, with basis given by t and [i,i]w (Example 1, §15(d)). Apply The-
orem 16.13 to obtain an isomorphism
T(x,y)l (x2 - \y) A H,(US2k;]k).
Then note that T(x,y)/ (x2 — \y) = T(x) = k[x], and that this isomorphism
sends i^a.
D
Exercises
1. Consider the graded vector space V — {Vi}i>o and its graded dual W =
Horn (K,Q). Let ??)9 denote the subgroup of permutations ? of the set {1,2,...,
? + q} such that:
?? < ?2 < · · · < ?? and ? (? + 1) < ? (? + 2) < · · · < ? (? + q) .
Define a product (called the shuffle product) on TW by:
[lui <g> W2 ® ¦ ¦ ¦ ® Wp] € TPW , [w[ ® iiig ® · · · ® I«,]
E/'_1V ??/) ??) ill ??) · · · ?? 7/) I
V L) \Wcl^>Wc2^ <& Wa(p+q)\
?€??,,
if 1 < ?? < ?
Here so denotes the graded signature. Prove that the coproduct defined on TV
in §3-exercise 4 dualizes the product * on TW.
2. Determine the algebra H,(QCPn;Q) for ? > 2.
3. Let X be a finite product of simply connected Eilenberg-MacLane spaces.
Determine the algebra H,(ClX; Q).
4. Let X be a simply connected homogeneous space. Prove that all triple
Whitehead products are trivial in ?*(?)
5. Determine, up through dimension 5, the Sullivan minimal model of X =
sl v Si U[ai[MMH, D6. Does the subalgebra H.(U(S2 V S2)); Q) generate the
algebra H, (QX;Q)?
6. Prove that, up through dimension 5, the Sullivan minimal model of S2\/S2\/S5
is given by ? ? = f\(x1,x2,X3,yi,y2,y3, zu z2,....) with V — {V/1} and Xi,x2 €
Km *3 € I/05, dVp C (AK)?!/, ctyi = x? .d^ = XiX2,dy3 = x\, dzx = xlV2 -
x2Ui ,dz2 = Xiy2 — x2y2 (see §13-exercise 4). Compute the Whitehead products
236 16 The loop space homology
[a,?}w = 0 for every ? € ?2E2 V S2 V S5) <g> Q and ? € ?3E2 V S2 V
Let D : F -» ? ? be a linear map such that D - d{Vi) C (AF)<i_2. ?
D extends to a differential on AV (cf. exercise 5).
7. Let V = Ikx ? Iky be the graded vector space generated by the
? € Vp and y € F,, p,c > 2. Prove that ((ifc ® V) ®T(sV),d), with
by cte = 0 = dy and for saisa^.-.sak € jTfe(sV), d(sa\sa2---sak) — &\ ®
is a r(sF)-semifree resolution of the field ife. Deduce that
isomorphic to the graded vector space T(sV).
17 Spatial realization
In \17(a), the ground ring is an arbitrary commutative ring, k. In the rest of
§i7, k is a field of characteristic zero. Occasionally we specify k = Q.
We began Part II with the construction, in §10, of the (contravariant) functor
Apl from topological spaces to commutative cochain algebras. Now, at the
conclusion of Part II, we reverse the process, and construct the (contravariant)
spatial realization functor
| | : commutative cochain algebras ~-> CW complexes.
Recall that a Sullivan algebra (AV, d) is simply connected of finite type if
V = {Vp}p>o and each Vp is finite dimensional. When k = Q the spatial
realization functor has the following important properties:
• Any simply connected rational Sullivan algebra of finite type is a Sullivan
model of its spatial realization:
(AV,d) -^ APL(\AV:d\) ,
and this spatial realization is a simply connected rational topological space
(Theorem 17.10).
• Any morphism ? : (AV, d) —> (AW, d) of simply connected rational Sulli-
van algebras of finite type is a Sullivan representative of its spatial realiza-
tion \?\ : \AV,d\ <— \AW,d\, A7.14).
• Two morphisms ?, ? : (AV, d) —> (AW, d) between simply connected ra-
tional Sullivan algebras of finite type are homotopic if and only if the con-
tinuous maps \?\ and \?\ are homotopic (Proposition 17.13 and §12(c)).
• Let ? : (AV, d) —> (AW, d) be a rational Sullivan representative for a
continuous map / : X —> Y between simply connected CW complexes with
rational homology of finite type. Then there is a homotopy commutative
diagram
X i Y
hy
\AW,d\ \AV,d\
\v\
in which ?*(/?.?) <S> Q and 7r*(/i>') ® Q are isomorphisms (Theorem 17.15).
From these properties it is easy to deduce (and we leave this to the reader)
the bijections
.. , , . ? ( isomorphism classes of ?
rational homotopy | ^ I ¦ ¦ ,?. n- 17 I
r —>¦ \ minimal Sullivan algebras >
1 J
1 over
and
238 17 Spatial realization
{homotopy classes of ^j ( homotopy classes of I
continuous maps of > -^ < morphisms of Sullivan algebras >
rational spaces J [ over Q J
promised at the start of §12. (Note that for these bijections we restrict to simply
connected CW complexes with rational homology of finite type and to simply
connected Sullivan algebras of finite type.)
The spatial realization functor, | |, is constructed as the composite of two
others. The first is Sullivan's simplicial realization functor [144]
{ ) : commutative cochain algebras -w simplicial sets ,
which is the adjoint of Apl(-), and the second is Milnor's realization functor
([126])
| | : simplicial sets -w CW complexes .
In describing Milnor's functor we shall only sketch some of the proofs, referring
to May's elegant exposition [122] for details.
We complete this section by using integration to define a natural quasi-isomorphis
C.{AV,d) -=>¦ Kom((AV,d),]k) ,
compatible with geometric products.
This section is organized into the following topics:
(a) The Milnor realization of a simplicial set.
(b) Products and fibre bundles.
(c) The Sullivan realization of a commutative cochain algebra.
(d) The spatial realization of a Sullivan algebra.
(e) Morphisms and continuous maps.
(f) Integration, chain complexes and products.
(a) The Milnor realization of a simplicial set.
Recall the definition of a simplicial set from §10(a), with face and degeneracy
maps di and Sj. Recall also that in §4(a) we introduced the geometric face and de-
generacy maps ?, = (e0 · · · ?, · · · en) : ?" —» ?" and q, = (e0 · · · e^e,· · - · e„) :
?"+! —> ??.
Now let ? be a simplicial set. Give each Kn the discrete topology. Then the
Milnor realization of ? is the topological space
\K\=
Sullivan Models 239
where ~ is the equivalence relation generated by the relations
diO ? ? ~ ? x XiX , ? € Kn+l, ? € ?"
and
Sj-? ? ? ~ ? x ?jX , ? € ifn, x € ??+1 .
This construction is functorial: if / : ? —> L is a morphism of simplicial sets
then / = {fn : Kn —> Ln} and the continuous maps fn ? id : Kn ? ?" —>
Ln ? ?" factor to define the continuous map
\f\:\K\->\L\.
Recall that the face and degeneracy maps of a simplicial set satisfy the com-
mutation relations A0.2). A simplex ? € Kn is degenerate if ? = SjT, some
r € AVi-i! otherwise it is non-degenerate. The set of non-degenerate n-simplices
is denoted NKn (cf. §10(a)).
Recall further that dAn = {j\i(An-1) and put ?" = ?" - dAn.
i
Lemma 17.1 [122] The quotient map ?? ¦ \\Kn ? ?" —> \K\ restricts to a
?
bijection
qK : U NKn ? ?" -> \K\ .
proof: Denote qk simply by q. If ? ? ? € Kn ? ?" choose the least k such that
q(a x x) = q(r x y) for some (r, y) € iv^ ? ?*1. Then r cannot be degenerate (if
?
r = Sjcj then r x y ~ ? ? ^y) and y € ? (if y = AjZ then ? x y ~ dir x ?).
Hence q is surjective. A tedious computation using the commutation formulae
A0.2) shows that q is injective. D
Suppose L C ? is a sub-simplicial set. If ? € Ln and ? = sjt, ? € -f^n-i then
r = djSj-r = (9,-? e ??-?· Thus it follows from Lemma 17.1 that \L\ C \K\. In
particular, recall from §10(a) that the n-skeleton of ? is the sub simplicial set
K(n) determined by
NKk k < ?
Thus \K(n)\ C\K\.
Proposition 17.2 [122] The Milnor realization \K\ of a simplicial set ? is a
CW complex with n-skeleton \K(n)\ and ?-cells the non-degenerate n-simplices
? € NKn. The attaching map for ? is the restriction of qK to {?} ? dAn.
proof: Let ? € Kn. The quotient map q : ]\Kn ? ?" —» \K\ satisfies q(a ?
?
???) = q(dia ? ?) and it follows that q restricts to a continuous map qa :
240 17 Spatial realization
{?} ? duJ1 —> \?{?— 1)|. Thus, because of Lemma 17.1. q induces a continuous
bijection
q(n):\K(n-l)\U{qa) ( ]J {?} ? ?" J -> \K(n)\ .
A straightforward check shows that the q(n) are proper, and hence homeomor-
phisms. Thus the q(n) define a continuous bijection to \K\ from a CW complex
with the desired properties. This is also proper, and hence a homeomorphism.
D
Example 1 |?[?]| = ?".
In §10(a) we introduced the simplicial set ?[?] whose fc-simplices are the linear
maps
(et0 · · -eik) : Ak —» ?", 0 < i0 < ¦ · ¦ < ? < ? .
The identity map of ?" is thus an ?-simplex ? of ?[?], and it is straightforward
to check that qA[n) restricts to a homeomorphism {i} ? ?" -^ |?[?]|. D
Example 2 Cones.
The 'simplicial point' is the unique simplicial set with a single fc-simplex cn in
each dimension. Now suppose ? is any simplicial set. We extend the face and
degeneracy maps in {Kn ]J{cn}}n>0 to the sequence of sets
{CK)n = Kn U H Kk X {Cn.u.,} U {Cn}
\fc=l /
by setting (for ? € Kk)'·
di{a,cT) = < ? ,if r = 0 and i = A: + 1.
^ (?, cr_i) , otherwise.
and
(?, cr+i ) , otherwise.
This defines a simplicial set CK, the cone on ?.
It follows now from Lemma 17.1 that \CK\ = \K\ ? ?/\?\ ? {1}. In particular,
• for any simplicial set ? the realization \CK\ of the cone on ? is a con-
tractible CW complex. D
Example 3 Extendable simplicial sets.
Recall from §10(a) that a simplicial set ? is extendable if for any ? > 1 and
any X C {0,. ..,n} the following condition holds: given ?* € A"n-i5 i € J and
satisfying <9*?,· = ?,-??*, i < j, then there exists ? € Kn such that 9?·? = ?,·,
i € I. We observe now that
Sullivan Models 241
• If K is an extendable simplidal set then \K\ is contractible.
Indeed, extendable simplicial sets ? have the property that if ? is sub sim-
plicial set of a simplicial set L then any morphism ? : ? —> ? extends to
a morphism L —> K. (This was proved in Proposition 10.4(ii) for simplicial
cochain complexes, but the argument applies verbatim to simplicial sets.)
In particular the identity of ? extends to a morphism ? : CK —> K. Thus
id\K\ = \?\ ? |?| where ? : ? —> CK is the inclusion. Since \CK\ is contractible,
id\K\ ~ constant map; i.e. \K\ is contractible. ?
Let ? be a simplicial set. Just as we defined the cochain algebra C*(K) in
§10(d) so we now introduce the differential graded coalgebra, C,(K), defined as
follows:
• C,{K) = {Cn(K)}n>0 and Cn{K) is the free lr-module on Kn divided by
the submodule spanned by the degenerate simplices.
• The comultiplication ? in C*(K) is given by
?
?? = ^ dpdp+i ¦ ¦ ¦ dna <g> d0 ¦ ¦ ¦ doa , ? € Kn .
• The differential d is given by
?
da = Y^{-l)pdpo , ? € Kn .
p=0
The reader will immediately see that this generalizes the construction in §4(a):
if X is a topological space then C»(X) = C*(S*(X)). Moreover C*{K) is the
cochain algebra Iiom(C*(K), k), exactly as in the case of topological spaces.
If ? is any simplicial set then the continuous map qx of Lemma 17.1 restricts
to continuous maps qa : {?} ? ?" —> \K\, ? € ??. This defines an inclusion of
simplicial sets,
?? : ?-* S.{\K\) , ??':?^???
where S*(—) denotes the simplicial set of singular simplices defined in §10(a).
Observe now that ?? maps the non-degenerate simplices of ? bijectively to
singular simplices of \K\ that are the characteristic maps for the cells. This
identifies ?*(??) as a cellular chain model in the sense of Theorem 4.18. In
particular, ?*(??) is an isomorphism. Note as well that
?.(??) : C.(K)-* C.(\K\)
is an inclusion, and identifies C*(K) as a sub dgc of C*(\K\).
242 17 Spatial realization
On the other hand, Let X be any topological space, and define a continuous
map U Sn(X) x ?" —)¦ X by sending ? ? y ?-4 o(y). This factors over the
?
quotient map qs.(X) to produce the continuous map
sx:\S.(X)\-*X.
It is an interesting but reasonable exercise [122] to show that sx is always a
weak homotopy equivalence. We shall limit ourselves here to the easy case that
X is a simply connected CW complex.
Proposition 17.3
(i) If X is a simply connected CW complex then s ? is a homotopy equivalence.
(ii) If ? is a simplicial set and \K\ is simply connected then \??\ is a homotopy
equivalence.
proof: (i) For simplicity put Y = \S*(X)\ and ? = CS.(X) : S,(X) —> 5,A').
We show first that Y is simply connected. Indeed, since X is connected any two
points x, y € X are connected by a singular 1-simplex / : (/,0,1) —> (X,x,y).
Thus any two 0-cells ?(?) and ?(?/) in Y are connected by the l-cell ?(/); i.e., ?
is path connected.
If g : A,0,1) —> (X, y, z) we define
i{2t) ^<?<?·
Similarly, f~l (t) = /(I -1). The homotopy class of / rel {0,1} is denoted by [/]
and an associative composition of homotopy classes is defined by [/]*[<?] = [/*<?],
— cf. §1.
Fix a 0-cell, ???, as a base point of Y. The Cellular approximation theorem 1.2
asserts that any loop in Y at ??0 can be deformed into the 1-skeleton ??. The
easy part of the Van Kampen theorem asserts that any element 7 6 ??(??,???)
can be written in the form [?/?]*1 * · · · * [?/?]*1 where the ?/j are 1-cells in Y.
There are obvious singular 2-simplices
and // \5 ? > X
const / * g
Sullivan Models 243
and ?? and ?? are singular 2-simplices in Y that provide homotopies ?/ ~
(?/)-1 rel {0,1} and ?/*?9 ~ ?(/ * 5) rel {0,1}. Let 7 = tf1 *¦¦¦* f±\ where
we have removed the (necessary) brackets for simplicity. Then / is a loop in A"
at x0 and our observations imply 7 = [?/]. Since AT is simply connected there is
a singular 2-simplex
const ? > X
const
and ?? is a homotopy from ?/ to the constant loop. Hence ??(?') = {0}.
Next recall that /7»(??·;?) is an isomorphism for any simplicial set K. In
particular, ?,(?;?) is an isomorphism. But the continuous map sx : Y —> X
induces S*(sx) : S,(Y) —> S*(X), and it is immediate from the definitions that
? : S»(X) —> S*(Y) satisfies 5»(??)? = id. Thus H,(sx;Z) is an isomorphism.
Since a homology isomorphism between simply connected spaces is a weak homo-
topy equivalence (Theorem 8.6) and a weak homotopy equivalence between CW
complexes is a homotopy equivalence (Corollary 1.7), assertion (i) is established.
(ii) Assertion (i) states that s\k\ : |5»(|/sT|)| —> \K\ is a homotopy
equivalence. It is immediate from the definition that s^ ? \??\ = id. Thus |^|
is a homotopy equivalence.
D
(b) Products and fibre bundles.
The product of two simplicial sets ? and L is the simplicial set ? ? L =
{{Kn ? Ln},di ? di,Sj ? Sj). If ? -^> ? <— L are morphisms of simplicial sets
then the fibre product ? ? ? L C ? ? L is the sub simplicial set defined by
(K xE L)n = {(?,?) | ?? = ??}.
Now the projections qk ¦ ? ? L —> ? and ql : ? ? L —> L are morphisms
of simplicial sets. Set
f = (\QK\,\QL\)'-\KxL\-*\K\x\L\.
Proposition 17.4
(i) The continuous map f is a natural homeomorphism \K ? L\ —* \K\ ? \L\.
(ii) Furthermore, f restricts to a homeomorphism \K Xe L\ -^ \K\ ?|?| \L\.
proof: We first introduce some notation. Given a surjective map
? : {?,.,.,?} —> {?,...,*} such that 0 = ?@) < ··· < ?(?) = k we put
244 17 Spatial realization
Qa — (eQ(o) " ' " ea(n)) '· ?" —> Ak. Then ?? is a composite of degeneracy maps:
?? = ??} ? · · · ? Qin_h, and in any simplicial set ? the map sa = Sin_k ? · · · ? s,a :
R'k —> A« depends only on a and not on the choice of decomposition (use the
commutation formulae A0.2)). Moreover the map qx of Lemma 17.1 identifies
saa ? y with ? ? Qay.
We now turn to the actual proof.
(i) It is immediate that / is proper and so we only need to show it is bijective.
We do so via Lemma 17.1. Any simplex in (? ? L)n has the form ? = (saa, spr)
with ? € NKp and r € NKg and it is degenerate precisely if sa = Sisa', and
S? = SiS?' for some {. This is equivalent to: a(i) = a(i + 1) and ?(i) = ?(i + 1).
Thus the non-degenerate simplices are the (sao, spr) with a(i) + ?(i) < a(i +
l) + /0(i + l), all*.
Now / : {?} ? ?" —> ({?} ? ??) x ({?} ? ??), where ? = ?(?,?) is the
? (? ?) ? °
dimension of ?. We denote this restriction by fa>? : ?" —'—> ?? x Aq- We
have to show that each fa? is injective and that
(a'0)), A7.5)
?,?
where the ?, ? run over all the non-decreasing sequences such that ? = (saa, S?r)
is non-degenerate.
Regard ?? ? ?? as a subset of W+1 ? W+1. Then fQi0 is the linear map
defined by e, ?-> (eQ(t). e^(,)), 0 < i < ?(?,?). Since either a(i) < a(i +
1) or ?(i) < ?(i + 1) it is easy to see that {ea(Q),e?@)) ,..., {ea{n),e?{n)) are
linearly independent in W+q+2. Hence each fa? is injective. Formula A7.5) is
a straightforward computation.
(ii) By definition / restricts to a continuous injection fs '¦ \K ? ? L\ —>
\K\ X|?| \L\ and this is proper because \K Xe L\ is closed in \K ? L\. We have
only to show that /# is surjective.
Suppose that ? = (saa, S?r) is a non-degenerate ?-simplex in ? ? L (as de-
o
scribed in the proof of (i)) and that y G ??· Then fqKxL&xy) = (??'(? x gay),
qh(T x Q?V))· Suppose this point is in \K\ X\e\ \L\· Then
??(?? ? Qay) = qE^ x Q?y) ¦
Write ?? = sa>o' and ?? — S?>r'', where ?' and ?' are non-degenerate in E. The
equation above gives
? ?
But ?? '· ?" —> ?? and similarly for Q?, ??< and Q?<. Thus Lemma 17.1
?
implies that ?' - ?' and ga'Qay - Q?'Q?y. Now y - ^ f,-et· and the condition
?
?
y G ?" means each ij > 0. It follows that the equation Qa<QQy — Q?'Q?y implies
245
Sullivan Models
Qa'Qa = Q?'Q?-, whence sasa' = S?Sp·. Thus ipsaa = saSa'&' = S?S?>r' =
i.e. ? € {? xE L)n and gxxz,(^ x y) € \K xE L\-
Recall from §2(d) that a fibre bundle with fibre ? is a continuous map ? :
X —> Y such that for some open cover {Ua} of Y we have: p~l{Ua) — Ua x Z,
compatibly with the projection on Ua.
There is a useful simplicial analogue of this "local product structure." Let L
be a simplicial set. As we observed in §10(a) any simplex ? € Ln determines
a unique simplicial map ?* : ?[?] —> L such that ?* (id^*) = ?. Thus given
a morphism of simplicial sets ? : ? —> L and ? € Ln, we can form the fibre
product ?[?] ? ?, ?.
We define a simplicial fibre bundle with fibre F to be a morphism ? : ? —> L of
simplicial sets such that for each ? > 0 and each ? € Ln there is a commutative
diagram
?[?] x F ^ ?[?] ??, ?
in which <?>? is an isomorphism of simplicial sets.
Remark Note that a simplicial fibre bundle is not a simplicial object in the
category of fibre bundles!
Proposition 17.6 If ? : ? —> L is a simplicial fibre bundle with fibre F then
\?\ : \K\ —> \L\ is a fibre bundle with fibre \F\.
proof: Recall from §17(a) that the cells of \L\ are the non-degenerate simplices
? of L and that the characteristic maps are the maps qa : {?} ? ?" —> \L\. It
is immediate from the definitions (cf. Example 1 of §17(a)) that
Thus the pullback of |?| to'an ?-cell is just the fibre product |?[?]| Xj^j \K\
defined with respect to |?,| : |?[?]| —> \L\ and \?\ : \K\ —> \L\.
Now apply Proposition 17.4 to translate the simplicial diagrams above to the
commutative topological diagrams
\A[n}\
246 17 Spatial realization
These show that \?\ pulls back to a product bundle with fibre |F| over every cell.
Thus Proposition 2.7 asserts that \?\ is a fibre bundle with fibre \F\. ?
Example 1 A simplicial construction of the Eilenberg-MacLane space K(k, 1).
Recall that k is our ground field of characteristic zero. Here we think of it
simply as an abelian group under addition. In §10(c) we introduced the simplicial
cochain algebra Apt = {{Api)n}. Thus each APPL is a simplicial vector space.
Let d be the differential in APL; then each (kerd)p is a sub simplicial vector
space of APPL. Put ? = (kerdI. We shall show that
• \Z\ is an Eilenberg-MacLane space, K(k,l).
For this let [k] be the simplicial vector space given by [k]n — k, di — id,
Sj = id. Since H((APL)n,d) = k,n> 0, (Lemma 10.7),
0 —>· [k] -)/????-»0
is a short exact sequence of simplicial vector spaces.
Let ?» : ?[?] —> ? be the simplicial map determined by any fixed ? € Zn.
We show that there is an isomorphism
??:?[?] ? [*] S ?[?] xZA0PL,
coherent with the projections on ?[?]. For this, fix r € (Apl)u satisfying dr = ?.
If ? G ?[?]& and ? € [k]k = k, define ??(?,?) = (?,?*(?) + ?). It is immediate
from the definition that ?? is a simplicial isomorphism.
We may now apply Proposition 17.6 to conclude that
|d| : \APL\ -> \Z\ A7.7)
is a fibre bundle with fibre |[ifc]|. The elements of [k]o (= k) are the only non-
degenerate simplices, and this identifies \[k]\ = k, equipped with the discrete
topology. (For example, if k = M. or Q, this is not the standard topology!)
Thus \d\ is a covering projection with discrete fibre, k. Since A°PL is extendable
(Lemma 10.7), I^J is contractible (Example 3, §17(a)).
Finally, note that addition in each (Apl)^ defines a simplicial morphism A°PL ?
APL —> A°PL, which restricts to a : A°PL ? [k] —> APL. This realizes to a
continuous map
\ct\:\A°PL\xh-*\A°PL\ .
In the same way addition defines a simplicial morphism [k] ? [k] —> [k], which
realizes to ordinary addition in k. Thus |a| defines an action of the additive
group of Jfc on \APL\ and this identifies k with the group of covering transfor-
mations of the covering projection A7.7). Since \APL\ is contractible it follows
that JcS7Ti(|Z|) and ??>2(|?|) = 0. Thus \Z\ is a K(k,l)·
It is useful to identify the isomorphism k -^ ???(|?|) explicitly. For this fix
as basepoints of \Z\ and l^lp^l the 0-cells zo and ao corresponding to the zero
Sullivan Models 247
elements of Zo and (APL)°. Note also that each ? € Jt = {???)°0 determines a
0-cell ax in 1.4^ |. Now recall (§10(c)) that (APLI = \(tudti). For each ? G k
the element Xdti € Z\ defines a 1-cell in \Z\ that is a loop g\ at zq. Its lift is
the 1-cell in \APL\ corresponding to ??? € {???)? and this is a path /? from uq
to ??. Thus
• The isomorphism h -^ ?? (|Z|,zo) is given by ? ?-> [g\\.
?
(c) The Sullivan realization of a commutative cochain algebra.
Recall again from §10(c) the simplicial cochain algebra (ApL,di,Sj). It may
be thought of as a sort of 'generalized point'. From this perspective the Sullivan
realization of a commutative cochain algebra (A, d) is analogous to the construc-
tion of an algebraic variety Vr from a noetherian commutative algebra R. The
points of Vr are just the algebra morphisms R —)¦ Ik. Analogously, we make the
Definition The Sullivan realization is the contravariant functor (A, d) n^ (.4, d)
from commutative cochain algebras to simplicial sets, given by:
• The n-simplices of (A,d) are the dga morphisms ? : (A, d) —> {Api)n.
• The face and degeneracy operators are given by d{O = d{ ? ? and s ja =
Sj ? ?.
• If ? : (A, d) —> (?, d) is a morphism of commutative cochain algebras then
{?) : (A,d) <— (B,d) is the simplicial morphism given by (?)(?) — ? ? ?.
?€(?,?)?-
Denote by DGA(—, —) and by Simpl(—, —) the set of morphisms between two
dga's, and between two simplicial sets. (Thus <^4, <i>„ = DGA ((A,d), {Api)n).)
Recall from §10(b) and §10(c) that if if is a simplicial set then Api(K) =
Simp^if, Apl) is the cochain algebra of simplicial morphisms from ? to Api-
Thus a natural bijection
OGA((A,d),APL(K))^Simp\(K,(A,d)) , ? >—> f , A7.8)
is defined by requiring
/(?)(?) = ?{?){?) , a 6 ?, ? 6 Kn, ? > 0 .
This establishes ???(—) and ( ) as adjoint functors between commutative
cochain algebras and simplicial sets. In particular, adjoint to the identity of
(A, d) is the canonical dga morphism
VA:(A,d)->APL(A,d) .
Combining Sullivan's functor with Milnor's realization gives the fundamental
248 17 Spatial realization
Definition The spatial realization of a commutative cochain algebra (A, d) is
the CW complex \A,d\ = \(A,d)\. The spatial realization of a morphism ? :
(A,d) —» (B,d) is the continuous map \<p\ — \{?)\·
Example 1 Products.
Let {A,d) and [B, d) be commutative cochain algebras. A pair of morphisms
? : (A,d) —> {Api)n and ? : (B,d) —> {APi)n extend uniquely to the mor-
phism ?"? : (A,d) <g> (B, d) —> (???)?· Thus restriction to A and restriction
to ? defines an isomorphism of simplicial sets
((A, d) ® {B, d)) A (A, d) ? (?, d) .
On the other hand, Milnor's realization converts simplicial products to topo-
logical products (Proposition 17.4). Thus we obtain a natural homeomorphism
\(A,d)®(B,d)\^>\A,d\x\B,d\ . ?
Example 2 Contractible Sullivan algebras have contractible realizations.
Suppose A = (A(U @dU),d) is a contractible Sullivan algebra, and let {ua}
be a basis of U. An element of (A)n is a morphism ? : A —> (Apl)u, and so an
isomorphism
is given by ? ?—> {???}. By Lemma 10.7 each (Apl)\u\ is extendable, and
hence so is the product (a trivial exercise). It follows (Example 3, §17(a)) that
\A\ is contractible. ?
More generally, we shall see now that spatial realization converts relative Sul-
livan algebras (§14) to fibre bundles with geometric fibre the realization of the
Sullivan fibre. (This is the obverse of the theorem in §15 that in the Sullivan
model of a fibration the fibre of the model is a model of the fibre.)
More precisely, fix a relative Sullivan algebra
\:{B,d) —>(?®AV,d)
such that Imd C (jB+®AV)e(A-®AF). Dividing by B+®W defines a Sullivan
algebra (AV,d).
Proposition 17.9 The continuous map |?| : \B (8) AV, d\ —> \B,d\ is a fibre
bundle with fibre \AV, d\.
proof: In view of Proposition 17.6 it is sufficient to identify (?) : E®AF, d) —>
/R ?\ as a simplicial fibre bundle with fibre {AV, d). In other words we need to
Sullivan Models 249
show that for any ? € (B,d)n the corresponding fibre product (with respect to
?, : ? [?] —> (B,d)) satisfies
?[?] x{BA) (? ® ? F, d) 9? ? [?] ? <AV, d) ,
compatibly with the projections on ? [?].
Now ? is a dga morphism (B, d) —> (APL)n. Moreover, for any ? € A[n]k, q
is a linear map ?*-' —> ?" and ?»(?) = APL(a) ? ?, as follows at once from the
definitions. Thus the &-simplices in the fibre product above are the pairs (a, r)
where ? € A[n]k and ? : {? <g> KV, d) —> {Api)k satisfy r ? ? = ???(?)?.
Let {{???)? <g>AF,d) = (.4pz,)n <S>b (B <g> AV'.d) be the pushout relative Sul-
livan algebra (§14(a)) defined via ?. Since (APL)n = .4Pi (?[?]) (§10(c)) there
is a unique simplicial morphism, ?[?] —>· ((-4pz,)n), adjoint to the identity of
(-4pi)n· Our observations just above identify
?[?] X(Bid) <?®AV,d> = ?[?]
compatibly with the projections on ?[?]. On the other hand, ? ((APL)n) = k
(Lemma 10.7). Thus the argument of Lemma 14.8, with k —> (APi)n replacing
(Bi,d) —> (B,d), shows that the identity of (Apl)u extends to a dga isomor-
phism (APL)n®(AV,d) = ((APL)n®AV.d). This identifies the right hand fibre
product as (?[?] xpPl)n) ((APL)n)) x (AV,d) = A[n] ? (AV,d). ?
(d) The spatial realization of a Sullivan algebra.
Fix a Sullivan algebra (AV, d). By definition. V = {Vp}p>i and so there is a
unique augmentation ? : (AV,d) —> k, which is then the unique 0-simplex in
(AV, d) and the unique 0-cell in \AV, d\. Denote all these by ?.
In §17(a) we introduced the inclusion of simplicial sets ? = ?(??,?) '¦ (?F, d) —>
5» (|AV,d|). Thus ???(?) is surjective (Proposition 10.4). As described there
-for integral coefficients, ?*(?;1?) is an isomorphism, and so ???(?) is a quasi-
isomorphism. Thus in the diagram
Apl((,
(AV,d) > APL((AV,d))
we may (Lemma 12.4) lift ?^?,?) through the surjective quasi-isomorphism
ApL^) to obtain a canonical homotopy class of dga morphisms
m(AV,d) ¦ (AV,d) -> APL (\AV,d\) .
We also want to compare Hom^V, Jc) with the homotopy groups ?, (\.\V, d\).
To do so we shall rely on the notation, constructions and observations of §13(c),
often without explicit reference, as well as to much of §12, which we also take
for granted here. Thus if ? : (Sn,x0) —> (\AV,d\,e) represents a G ?? (\AV,d\)
250 17 Spatial realization
then Apl (a) ° m^v,d) lifts to a morphism ?? from (AV, d) to the minimal model
of Sn, which has the form (A(e),0) or (A(e, e'),de' — e2) with dege = n.
Furthermore, ?? restricts to a linear map Vn —> he which depends only on
a, and hence determines a map
( ; ) :Vn ??? (\AV, d\) —>¦ k , ??? = (?; a)e .
These maps are linear in Vn and, if ? > 2, additive in ?? (|AV, d\). Thus the
maps
C« :7rrt(|AV,rf|) -»· HomA(^A) , ??(?)(?) = (-1)>;?> ,
are morphisms of abelian groups if ? > 2.
Theorem 17.10 Let (AV, d) be a Sullivan algebra such that Hl(AV,d) = 0
and each Hp(AV,d) is finite dimensional. Then
(i) \AV,d\ is simply connected and ?? : 7rn(|AV,d|) -^ Romjk(V.It) is an
isomorphism, ? > 2.
(ii) If 3c = Q then m(Av,d) 25 ? quasi-isomorphism,
m{AV,d) : (AV,d) -^ APL (|AV,d|) .
When Jc = Q then Theorem 17.10 exhibits any simply connected Sullivan
algebra of finite type as the Sullivan model of a CW complex.
proof of Theorem 17.10: We first reduce to the case (AV,d) is minimal.
Use Theorem 14.9 to write (AV,d) = (AW,d) ® (A(U ®dU),d) with (AW,d)
minimal. Then |AV,d| is the product of \AW, d\ and a contractible CW complex
(Examples 1 and 2, §17(c)). It is thus sufficient to prove the theorem for (AW, d).
We may therefore assume that (AV, d) itself is minimal. This implies that V =
{Vp}p>2 and that each Vp is finite dimensional.
We shall rely on the following observation. Suppose (AU,d) —> (AU <g>AV,d)
is a minimal relative Sullivan algebra in which (AU, d) is itself a minimal Sullivan
algebra. As in Proposition 17.9 this realizes to a fibre bundle \AU (8) AV,d\ -^
\AU, d\ with fibre |AV, d\. In particular, |?| is a Serre fibration (Proposition 2.6).
Let dt : ?, (\AU,d\) —> ?«_? (\AV, d\) be the connecting homeomorphism.
Next, by adjointness we have the commutative diagram
(AU,d) > (At/®AV,d) " (AV,d)
APL ((AU,d)) — ApL ({AU <8) AV,d» — APL ((AV,d))
Sullivan Models
251
Use the Lifting lemma 14.4 to choose m(Ai/id), m.(,\u®AV,d) and m(AVj) so that
the diagram
(AU,d)
(AU <g> AV, d)
(AV, d)
ApL(\AU,d\) — APL(\AU®AV\,d) — AF
commutes.
Now the argument of Proposition 15.13 establishes that
(???;?) = (-?)?+?(?;3,?) , ? G Vn, a 6 nn+1(\AU\) .
This translates to the commutative diagram
nn+l(\AU,d\) d' ' 7rn(\AV,d\)
A7.11)
(Un+\k) —f ?????(??,^,
in which d*0 is the dual of d0 : (d*of) (?) = (-l)n+1 f (???) ,? G Vn.
proof of (i): We turn now to the proof of (i), which is accomplished in stages.
Step 1: If deg ? = 1 then |?(?),0| is an Eilenberg-M acLane space K(k, 1), and
?\ is an isomorphism.
Recall that (A(v),0)n consists of the morphisms ? : (?(?),?) —> (APL)n.
Thus ?? G (kerd)Jj. Since the morphism ? is determined by ??, an isomorphism
of simplicial sets (?(?),?) ^» (kerdI is given by ? ?-*· ??. Thus our assertion
follows at once from the Example of §17(b).
Step 2: If deg ? — ?, ? > 2, then |?(?),0| is an Eilenberg-M acLane space
K(k,n), and ?? is an isomorphism.
Consider diagram A7.11) specialized to the case of the contractible Sullivan
algebra (?(?, w), dw = ?), regarded as the relative Sullivan algebra (?(?), ?) —>
(?(?,??),?). Then dp is an isomorphism by inspection, O« is an isomorphism
because |A(u, w),d\ is contractible (Example 2, §17(c)) and
Cn-i -" ??_! (|?(??),0|) —> Rom(kw,k) = k is an isomorphism by induction.
(Start the induction with Step 1!)
Step 3: Assertion (i) holds if V is finite dimensional.
We establish this by induction on dim V, the case dim V — 1 being Step 2.
Write V = U © Jkv so that (AU, d) is a sub-Sullivan algebra and ?? G AU.
252 17 Spatial realization
Thus (AU, d) —y (AU ®A(v),d) is a relative Sullivan algebra. Since (AV,d) is
minimal, dQ = 0. By induction on dim V, ?, : fr»(|Ai/,d|) —> Hom(U,k) is an
isomorphism. By Step 2, so is ?, : ??»(|?(?),0|) —> Hom(kv,k). Thus diagram
17.11 shows that d. = 0 : nm(\AU,d\) —> ?^^?^,?]).
Now (by naturality) we have the row exact commutative diagram
0 — ??.(\?(?),0\) - 7T,(|AV',d|) 7r.(|At/,d|) — 0
0 — Eom(kv,Jc) ????(?',*) Eom(U,k) —~ 0
and it follows that the central arrow is an isomorphism.
Step 4: Assertion (i) is true in general.
Fix ? and let U = Vr^n+1. Thus we have a fibration |AV,d| —>· \AU,d\ with
fibre |AV">n+1,dj. Since (Api,)r is concentrated in degrees < r it follows that
for r < ? + 1 the only morphism (AV>T1+1,d) —> (Apl)j. is the augmentation
(AV>n+i,d) —>· k. Thus |AV>n+1,rf| has a single zero cell, and no r-cells for
1 < r < ? + 1.
It follows that ?4 (|AV>n+1,d|) = 0, 1 < r < n+ 1, and hence 7rn(|AV,d|) -^>
¦nn(\AU,d\). Moreover Un = V and ?? : nn(\AU,d\) -=»· Hom(t/n,A) is an iso-
morphism by Step 3. Hence ?? : 7rn(|AV,d|) —> Hom(Vn, k) is an isomorphism.
proof of (ii): It follows from (i) that |AF, d\ is simply connected and that each
7Tn|AV, d\ is a finite dimensional rational vector space, and that ?* : nr(\AV, d\) -^
Homij(T/r, Q) is an isomorphism. We again proceed in stages.
Step 1: V = Vn.
Here 7r,(|AV, 0|) is concentrated in degree ? and so |AV,d| is an Eilenberg-
MacLane space of type ?(?,?) with ?? = HomQ(V,Q). In particular, the
Hurewicz homomorphism is an isomorphism, hur : 7rn(|AV, 0|) -^ Hn(\AV, 0|;Z)
by Theorem 4.19. It follows that Hn(\AV,0\;Z) is a rational vector space. Alto-
gether then, since V" is finite dimensional, we obtain
V = HomQ(HomQ(F, Q),Q)
S Eomz(Hn(\AV..0\;Z),Q)
= Hn(\AV,0\:Q) .
Back-checking through the definitions identifies this isomorphism as the linear
map Hn (m(Al/0)) : Vn —>· Hn (.4PL(|AF, 0|)). Now it follows from Example 2
of §15(b) that m(AVi0) : (AVn;0) —> -4pL(|AVrn,0|) is a quasi-isomorphism.
Step 2: V = V^n.
Sullivan Models
253
Here we argue by induction on n, the case ? = 2 being covered by Step 1. Put
U = V<n. The naturality of 77 : (AV,d) —> APL((AV,d)) means that
(AV,d)
(AU ®AVn,d)
APL{{AU,d)) APL((AV,d))
commutes. Thus it is easy to arrange that
(AU,d) (AU®AVn,d)
APL(\AU,d\)
APL(\AV,d\)
APL((AV",O))
(AFn,0)
APL(\AVn,0\)
commutes as well.
In this diagram m^i/,^) is a quasi-isomorphism (induction on n) as is rn(\vn,o)
(Step 1). Thus Proposition 15.6 gives a quasi-isomorphism
m : (AU ® AVn, D) -=* APL(\AV, d\)
which extends m^\utd) and induces m(AK",o)· By the Lifting lemma 14.4 we
can extend the identity of (AU, d) to a morphism ? : (AU <g> AVn,d) —> (AU ®
AVn, D) such that ??? ~ m{AV4) rel (AU, d). Then (? - id) : Vn —>¦ At/, which
implies that v? is an isomorphism and ??^?,?) is a quasi-isomorphism.
Step 3; The general case.
For the general case we fix any ? and show that Hn (m\v,d) is an isomor-
phism. As observed in Step 4 of (i), \AV>n+l,d\ is (n+l)-connected. Thus it has
a Sullivan model of the form (AW>n+l,d), while \AV^n+l,d\ has (AV^n+1,d) as
its Sullivan model. By Proposition 15.6, |AV, d\ has a Sullivan model of the form
(??/<"+?(8)??^>?+1,?>) and hence Hn (\AV^n+1,d\;Q) A Hn (\AV,d\;Q).
This identifies Hn (??,(??,?)) with Hn (??^??<?+?^), which is an isomorphism
by Step 2. ' D
When It — Q and (AV, d) is a Sullivan algebra satisfying the hypotheses of
Theorem 17.10 then the quasi-isomorphism
) ^ APL(\AV,d\)
is called a canonical Sullivan model.
Now let X be any simply connected CW complex with rational homology of
finite type, and let
mx : (AW, d) -"'
254
17 Spatial realization
be a minimal Sullivan model. Since Apl(X) = Apl E«(JY)), the adjointness
formula A7.8) produces a natural simplicial map
-yx : S.(X)-+(AW,d) ,
adjoint to ?? ?. On the other hand in Proposition 17.3 we established a natural
homotopy equivalence s ? : \S*(X)\ —> X- Let tx be the inverse homotopy
equivalence (defined uniquely up to homotopy) and set
hx =
tx : X —» \AW,d\ .
Theorem 17.12 With the notation and hypotheses above,
(i) The diagram
APL(AW,d) .A"-{iw
APL\AW,d\ ApL{hx) . APL(X)
(AW,d)
is homotopy commutative.
(ii) If Ik = Q then all the morphisms in the diagram are quasi-isomorphisms.
In particular, hx is a rationalization of X (§9(b)).
proof: (i) The left hand triangle commutes by construction. Next observe that
S,(sx) °?s.(X) = id : S*(X) —>· 5,(Z). Since txsx ~ id]Sx\ we can apply
Proposition 12.6 to conclude that
?-PL
° ?
?-PL
? ApL(tx) ? ?
for any morphism ? : (AW.d) —>· APL (\S.(X)\). Thus
ApL(hx) ? m{AW4) ~ ApL (is-(X)) ° apl {\lx\)
the last equality following from the adjointness of •yx and ???.
(ii) According to Theorem 17.10, m^w,d) is a quasi-isomorphism. Hence
(h). In §17(a) we obser\'ed that ?*(??) is a quasi-isomorphism for
so is
Sullivan Models 255
any simplicial set K. In particular Apl (?(?\?,?)) iS a quasi-isomorphism. Hence
so is ?(?\?,?)·
Finally since \AW, d\ is a simply connected rational space and since Ht(hx;Q)
is an isomorphism (because Api(hx) is a quasi-isomorphism) it follows that hx
is a rationalization (Theorem 9.6). ?
(e) Morphisms and continuous maps.
In §12(c) we observed that homotopic continuous maps /o ~ /i : X —> Y
have homotopic Sullivan representatives. In the reverse direction we prove
Proposition 17.13 Iftp0 ~ ?\ : (AV,d) —> (AW,d) are homotopic morphisms
between Sullivan algebras then
\??\~\??\:\???,?\-+\??,?\.
proof: A homotopy from ?0 to ?? is a morphism ? : (AV, d) —> (AW. d) <g>
A(t,dt) such that (id®Si)<b = ??, where ?,(?) = i. Since realization preserves
products (the Example of §17(c)) we obtain a continuous map |?| : \AW, d\ ?
|A(i,di)l —>· |AV,d| such \?\(?,?{) = \??\{?). But the identity map of A(t,dt) is
a 1-cell in |?(?,dt)\ joining e0 to ??, and so \??\ ~ \??\. G
Now suppose ? : (AV,d) —> (AW,d) is a morphism of Sullivan algebras. By
adjointness — cf. A7.8) —
APL({AW,d)) ,
where ? continues to denote the adjoints of id(Av,<f) and id(t\w,d)- It follows that
(AW, d)
Av,.d, A7.14)
APL(\AV,d\) ^-— APL(\AW,d\)
is homotopy commutative. Thus if ? is a morphism of simply connected rational
minimal Sullivan algebras of finite type then A7.14) exhibits ? as a Sullivan
representative of \<p\.
Next, suppose / : X —> Y is a continuous map between simply connected CW
complexes with rational homology of finite type. Let ?? ? : (AW, d) —> APi(X)
and ??? : (AV,d) —> Apl(Y) be minimal rational Sullivan models, and recall
the continuous maps hx and hy defined at the end of §17(d).
256
17 Spatial realization
Theorem 17.15 With the hypothesis and notation above, let ?
(AW,d) be a Sullivan representative for f. Then the diagram
(AV, d)
X
S
Y
\AW,d\
\AV,d\
is homotopy commutative.
proof: In the notation at the end of §17(d), ?.? and ty are homotopy inverses
to sx and sy. Since fsx = sy|5,(/)| it follows that tyf ~ |S,(/)|?.v· But
hx = \jx\ ? tx, and so it is sufficient to prove that \??\ ? |S*(/)| ~ \?\ ? \jx\.
Because ? is a Sullivan representative of / there is a cochain algebra mor-
phism ? : (AV,d) —> APL{X) ® A{t,dt) such that (id®eo)$ - ApL(f)my
and (id <8>?i)i' = ????. Compose this with the obvious morphism Apl(X) ®
A(t,dt) —> Apl{S*{X) ? ?[1]) and take the adjoint morphism ? : S*(X) ?
?[1] —>· (AV,d) via the adjoint relation G.8). Then \?\ : brS,(/)| ~ \???\- U
(f) Integration, chain complexes and products.
Suppose given a Sullivan algebra (AV, d) and recall the natural morphisms of
cochain complexes
(AV,d) A APL(AV,d) ? C*{AV,d)
defined respectively in §17(c) and in §10(e). We denote by C(AV,d) the chain
complex Hom(AV, h). Then the composite § ? ? dualizes to a natural morphism
of chain complexes,
via the obvious pair-
(since C(AV,d) includes naturally in Hom(C*(AV,d),.
ing)·
Proposition 17.16 Suppose (AV, d) is a Sullivan algebra in which each V"
is finite dimensional and either V = V-2 or else d preserves V. Then J^ is a
quasi-isomorphism.
proof: Theorem 10.15(ii) asserts that / is a quasi-isomorphism. On the other
hand, so is any canonical Sullivan model, m^v.d)· (If V - ^-2 this is Theo-
rem 17.10. Ifd: V —y V a trivial modification of the proof gives the same result.)
Since ? = Apl{Q o"i.(Av,d) it follows that ? is a quasi-isomorphism too (§17(d)).
Thus the composite dualizes to a quasi-isomorphism Horn (C*(AV, d),lk) -^>
Sullivan Models 257
Finally, if C is any chain complex there is a natural morphism
C —> Hom(Hom(C, Jc),Jz). If C itself has finite type this is an isomorphism. If
H{C) has finite type then this is a quasi-isomorphism because H (Hom(—, k)) —
Horn (#(-),*). In the case of (AV,d) we have H*(AV,d) = H(AV,d) and so
H,(AV,d) has finite type. Thus since C*{AV,d) = Hom(C.<AV,d),lfc) the in-
clusion ? : C»(AV,d) —> Horn (C*(AV,d),]k) is a quasi-isomorphism too. Hence
so is the composite Jt — Horn (^ ? 77,1c) ? ?. ?
The quasi-isomorphisms §? : Apl(K) —> C*(K) are not a morphisms of
cochain algebras. In compensation, we show that the quasi-isomorphism ft is
'compatible' with geometric products.
For this we recall the definition (§17(b)) of the product ? ? L = {Kn ?
Ln, di x di, Sj ? Sj} of simplicial sets. The simplicial diagonal ?# : ? —> ? ? ?
is the morphism ? \—>¦ (?,?). Now in §17(a) we introduced the dgc, C,(K) and
identified it as a sub dgc of C»{\K\) via the inclusion ?*(??)- In this setting,
the Eilenberg-Zilber and Alexander-Whitney morphisms restrict to morphisms
c„(uq ® c.(L) ^^> c»(ir ? L) -^ c.(ur)
and the comultiplication in C»(K) is just AI-VOC^A^). Clearly AWoEZ = id
and tedious but straightforward computations show, as in Proposition 4.10 that
EZ ? AW ~ id.
Now suppose (AV, d) and {AW, d) are Sullivan algebras. A natural inclusion
C(.\v,d) ® C(AW,d) —)¦ ^(??,??)®(???,??) is given by
(? ®?)(? <g> ?) = (—l)degi> ??8??(?N(?), ?€ C(Av.d) ,
o € C(AW,<f); moreover this inclusion is an isomorphism if either AV or AW has
finite type. Recall also from the Example of §17(c) that we identify ((AV, d) ®
{AW,d)) = (AV,d) ? {AW,d).
Proposition 17.17 If {AV, d) and (AW,d) are Sullivan algebras then the dia-
gram
? (AV, d) ® ? (ATV, d) ^— ? ((AV.. d) ® (AIV, d))
commuies.
proof: First consider arbitrary simplicial sets ? and L and let ?? : ? ? L —y
? and pL : ? ? L —)¦ L be the projections. If ? G Apl(K) and ? G .4/»L(L) we
abbreviate Apl(jpk)$ AApl^Pl)^ to ? ? ?. We begin by showing by induction
onp + q that:
258 17 Spatial realization
• For any ? € APPL(K), ? € A9PL(L), ? € Kk and ? € Le,
A7.18)
Indeed, if ? + q = 0 then ? and ? are \'ertices, ??(? <g> ?) = (?, ?) and
the left hand side is just ?(?)?(?). This is (trivially) the same as the right
hand side. Suppose ? + q = ? and that the proposition is pnwed for ? — 1.
Recall from §10(a) that ? and ? determine simplicial maps ?» : ? [?] —>¦ /<"
and ?» : ? [g] —)¦ L. By naturality we may use these to reduce to the case
? = ? [?], ? = A[q]. Thus .4PL(A') = (APL)P and APL(L) = (APL)g, as
observed in Proposition 10.4(i). In particular there are polynomials f(t\,..., tp)
and g(ti,... ,tq) such that ? = /dti A ¦ ¦ · ? dtp and ? = gdt\ A ¦ ¦ ¦ A dtq.
Now either ? > 0 or q > 0. In either case ?? = ??? = 0. If ? > 0 clearly
? = dF and ? ? ? = ??(? ? ?), some ? G (^??,)^. Since §KxL commutes with
the differentials, for any c C. Cn(K ? L) we have
) (de) .
Lr ? ?
Since EZ also commutes with differentials we may substitute ??(? <S> ?) for c
and use induction to complete the proof. If ? = 0 and q > 0 write ? = du and
use the same argument. ( A second proof may be constructed starting from the
observation // f(t)g(s)dtds = ( / f(t)dt) ( / g(s)ds). This idea, while
JJxxY \Jx / \Jy J
more intuitive, is technically more complicated to implement.)
Now specialize to the case ? = (AV,d) and L = (AW,d), and observe that
? € W
? ® *) = *7(AV,d)* X ^(AW'.d)*, ? "'
Thus for ? e K,r€ L,
??(? ® ?) J (? ® ?) = ±( fKxL(vlAV,d)*
?® / ? J (?® ?) .
Exercises
1. Let (AV, d) be a Sullivan minimal model with dim H*(AV, d) < oo and F1 = 0.
Show that (AV,d) is the Sullivan minimal model of a 1-connected finite CW
complex.
Sullivan Models 259
2. Prove that the geometric realization of the relative Sullivan model (Ax2 <8>
Ax3;dx3 = x'l) is the rationalization of the principal fibration S3 —> S2 —>
K(Z,2).
3. Let (?(?3,?5),0) <g> (A(v3,v'3,v5,V7,v'7,U9,V9,w9,...) ,d) be the Sullivan min-
imal model of the product (S3 x S5) x (S3 V S3) . Compute the differential
d for the given generators. Consider the relative Sullivan model (A(x3,y5) ®
?(?3,?3,?5,?;7,?;7,??9,?9, wg, ...),D) with ? = d up to degree 8, Dug = dug,
Dvq = dvg and Dwg = dw9 + xsv5, ... . Prove that the geometric real-
ization of this model is rationally equivalent to the total space of a fibration
(S3 V S3) —> ? —> (S3 x 5°) with non trivial connecting morphism. If a and b
are generators of 7r3E3 V S3) compute [[?,
4. Prove that if ? : (A,dA) —> (B,d?) is a quasi-isomomorphism then ?*(\?\) :
H*(\A,d/i|) —> H*(\(B,cIb\) is an isomorphism.
5. Let ? : (At, 0) —>· A(x,y,z) be as in §3-exercise 3. Is \?\ an homotopy
equivalence?
6. Let (AV, d) be a Sullivan minimal model and ? an element of degree ? .
Prove that [(Ax,0),(AV,d)j = Hn(AV,d). Deduce that if X is a space then
[X,K(Q,n)}=Hn(X;Q).
Part III
Graded Differential Algebra
(continued)
18 Spectral sequences
The ground ring in this section is an arbitrary commutative ring ]k, except in
topic (d) where h, is a field.
Although we have managed to provide the reader with relatively simple 'spec-
tral sequence-free' proofs in Parts I and II, subsequent material necessarily uses
spectral sequences in a fundamental way. We therefore introduce them here, but
limit ourselves to the strict minimum that will be required. In particular, we
consider only the spectral sequence of a filtered object, and even there make no
attempt to present results in their most general form.
A spectral sequence is, in particular, a sequence of differential graded modules
(El,d') such that H(El) = El+l. They can be used to 'approximate' the homol-
ogy of a complicated differential module and in this (and other), contexts play
an essential role in homological calculations.
In this section we provide a brief and elementary introduction, including only
material that will be required in the following sections. In particular, much of
what is presented here holds in considerably greater generality.
This section is divided into the following topics.
(a) Bigraded modules and spectral sequences.
(b) Filtered differential modules.
(c) Convergence.
(d) Tensor products and extra structure.
(a) Bigraded modules and spectral sequences.
Recall (§3) that a graded module M is a family {M{};6z of modules. Anal-
ogously a bigraded module is a family M = {jV/i,j}({j)ezxz of modules indexed
by pairs of integers (i,j). The basic constructions of §3 (e.g. Horn and <8>) carry
over verbatim to the bigraded case. In particular a linear map / : M —> ? of
bidegree (p, q) is a family of linear maps / : Mij —> Ni+pj+g and a differential
is a linear map d : M —y M such that d2 = 0 and d has bidegree (—i, i - 1) for
some i. The homology, H(M) = kerd/lmd, is again a bigraded module.
Associated with a bigraded module M is the graded module Tot(M) defined
by
Tot(M)fc = ? Mitj
and a differential in M induces an ordinary differential in Tot(M).
Finally, as in the singly graded case, we raise and lower indices by setting
MiJ = Af-f.-j .
We can now define spectral sequences.
Graded Differential Algebra 261
Definition A homology spectral sequence starting at Es is a sequence
(Er,dr,ar)r>s
in which ET = {Ep q] is a bigraded module, dr is a differential in Er of bidegree
(—r, r — 1) and ?? : H(Er) -A· ??+1 is an isomorphism of bigraded modules.
A cohomology spectral sequence is a sequence (Er,dr,ar), r > s, with ET —
{Ep>q}, dr a differential of bidegree (r, -r + 1) and ?? : H{Er) A ?r+1. Thus
raising degrees converts a homology spectral sequence into a cohomology spectral
sequence.
We shall frequently suppress the ?? from the notation and refer to the spectral
sequence (Er,dr) or (Er,dr).
In calculating with (cohomology) spectral sequences it is frequently useful to
represent them by diagrams of the form
r, q - r + 1)
A morphism of spectral sequences is a sequence of linear maps ?^ : E^ —> E^
of bidegree zero, commuting with the differentials, and such that ?? identifies
? (?^) with <^r+1\ (or ?^) : E(r) —> E(r) such that ?? identifies ? (?(?))
With ?(?+1))·
(b) Filtered differential modules.
A filtered (graded) module is a graded module, M, together with an increasing
sequence
d----CFpcFp+1C·-- ,p<=Z
of submodules, called the filtration of M. (If M = {Mn} we write Fp = F_p
and so the filtration has the form
5 : - · ? Fp D Fp+1 D ¦ ¦ · .)
We may also write FPM instead of Fp if necessary to avoid confusion. The
filtered module (M, ?) determines the associated bigraded module, CM, given by
>P+4
= (Fp/Fp+l)
V+Q
262 18 Spectral sequences
Here p is called the filtration degree and q is the complementary degree.
A linear map ? : M —> ? between filtered modules is filtration preserving if
it sends each FP(M) to FP(N). In this case it induces Qtp : QM —> QN in the
obvious way. A filtered differential module (M, d, 5) is a filtered module (M, 5)
together with a filtration-preserving differential in M.
Example 1 Semifree modules.
Suppose (M, d) is a semifree (R, d)-module. Then (by definition, cf. §6) M
admits a filtration
0 C M@) C M(l) C · · ·
such that
(QM,Qd)^(R,d)®(V,0) .
D
Example 2 Relative Sullivan algebras.
Let (B ® AV, d) be a relative Sullivan algebra (§14). It is filtered by the degree
ofB:
? = 0,1,2,...
and the associated bigraded module is given by
(C(B ® AV), Gd) = (?, 0) ® (AV,d) .
Example 3 The word-length filtration of a Sullivan algebra.
Filter a Sullivan algebra (AV,d) by its word-length (cf. §12):
? > 0 .
The associated bigraded module is then given by
where do is the linear part of d : do : V —> V and d — do : V —> A-2V. D
Suppose (M, d, $) is a filtered (graded) differential module. Then (QM, Cd) is
a differential bigraded module in which Qd is a 'first approximation' to d. The
spectral sequence associated with (M, d, ?) is a spectral sequence beginning with
(GM, Qd) and, as we shall see in (c), 'converging' in many cases to H(M, d). We
shall define the cohomology spectral sequence for M = {M*}i6z; the homology
spectral sequence for M = {Mj}i6z is obtained simply by lowering indices.
To begin, denote the filtration of M by · · · D Fp D Fp+I D ¦¦¦ . Note that
each Fp is a graded module: Fp = {(Fp)n}neZ. For each integer r > 0 define
graded modules Zp and Dp by
Zp = {x€ Fp\dx?Fp+r} and Dp = Fp ? dFp~r .
Graded Differential Algebra 263
Evidently
D% C D{'C ¦·' C Dv C ··- C Z*r C ¦¦ ¦ C Z* C ?? .
Now define bigraded modules Er, r > 0, by letting Ef>q be the component of
Z?/ (Zpr±{ + Dpr_^ in degree ? + q:
It is immediate from the definition that d factors to define a differential dr in Er
of bidegree (r, 1 —r). Moreover, the inclusions Z^+1 c—> Zp induce isomorphisms
of bigraded modules
Er+i A H(Er, dr) .
Note as well that
Definition The spectral sequence (Er, dr) is called the spectral sequence of the
filtered differential module (A/, d, J).
Note that this construction is natural: if ? is a morphism of filtered differential
modules it induces a unique morphism ??(?) of spectral sequences such that
??(?) = ??.
Example 4 Bigradations.
There is one particular situation that arises frequently in practice. We suppose
given a graded differential module (M, D) in which
• Each Mr is given as a direct sum Mr = ? Mp'q.
p+q=r
• The differential d is a direct sum D = ?, A with Dt : ??? —? Mp+i'q~i+1.
i>0
Here a canonical filtration is given by Fk(M)r = 0 Mp'r~p and this leads as
p>k
just described to a spectral sequence.
Moreover from D2 = 0 we deduce D$ = 0, DXDQ + DODX = 0 and D'{ =
-DqDz + D2D0. In particular, Do is itself a differential and Dx induces a
differential ?(??) in H(M,D0). Now a straightforward verification shows that
the first two terms of the spectral sequence are given by
(Eo,do) = (M,D0) and (Eudx) = (H(M, A>), H(DX)) . ?
(c) Convergence.
Suppose (Er,dr) is a cohomology spectral sequence. Fix a pair (p, q). If the
maps
264 18 Spectral sequences
are both zero then H™ (Er, dr) = E™ and ?? : ?™ A ???. In this case
we write Epg = ?y+i· ^he spectral sequence (Er,dr) is called convergent if for
each (p, q) there is an integer r(p, q) such that
In this case the bigraded module E™ is defined by E™ = E™, r > r(p, q). The
spectral sequence collapses at Er if di = 0, i > r. In this case E^ is defined and
Er = Eco.
An important example of convergence arises in the case of first quadrant spec-
tral sequences, which we now define.
A first quadrant cohomology spectral sequence is one in which each ET =
{E%'q}p>0 q>0. These spectral sequences are convergent with E™ = E™, r >
max(p, q + ?). Analogously, a first quadrant (cohomology) filtration in a graded
module M is a filtration {FPM} such that
F°M = M and FPM = {(FpM)"}n>p .
Notice that these conditions imply that M = {M"}„>0.
Let (M, d, ?) be a filtered differential (graded) module. Define a filtration in
H{M) by
FP(H(M)) = Im (H(FpM) —»· H{M)) .
If the associated spectral sequence is convergent and if there is a natural isomor-
phism Eoo = QH(M) then we say the spectral sequence is convergent to H(M).
Proposition 18.1 Suppose (M, d, #) is a cochain complex with a first quadrant
filtration. Then the associated spectral sequence is first quadrant,and converges
to H(M).
proof: The first assertion is immediate since Eq = QM is necessarily concen-
trated in non-negative bidegrees.
For the second assertion let Zpq be the component of degree ? + q in the
graded module Zp. If r > q + 1 then
d : Z™ —»· (Fp+rM)p+q+1 = 0 .
Thus
Zp'q = kerdr\(FpM)p+" and, similarly, ??±\>"-1 = kerdn (FP+1M)P+Q .
Moreover, if r > ? then Dpr_x = d{M) ? FPM. This identifies
p'" = zp>y (zprt\>q-1 + (^_!)P+9) = gp>*H(M). a
Graded Differential Algebra 265
Proposition 18.2 (Comparison) Let ? : (M,d,$) —>· (N,d,$) be a mor-
phism of filtered cochain complexes with first quadrant filtrations. If some ??(?)
is a quasi-isomorphism then ? {?) is an isomorphism of filtered modules. In
particular, ? is a quasi-isomorphism.
Lemma 18.3 Suppose ? : (M, 5) —> (-W>5) is a morphism of filtered graded
modules with first quadrant (cohomology) filtrations. IfC(ip) is injective (resp. sur-
jective) then so is ?.
proof: Suppose Q(ip) is injective. If 0 ? x 6 M" there is a greatest ? such
that ? € FPM, because (Fn+1M)n = 0. Thus ? represents a non-zero element
[x] € Qp'n~p(M) and so 0 ? Q(rp)[x] = [??]. In particular ibx ? 0.
Suppose G(ip) is surjective. Then for each p, ip(FpM) + FP+1N = FPN. It
follows by induction on q that ? (FPM) + Fp+qN = FPN, q > 0. Fix ? and
choose q so p + q > n. Then (Fp+qN)n = 0. It follows that ? (FPM) = FPN. ?
proof of 18.2: Since E{+\ (?) is identified with ? (?{(?)) it follows that Er+i(<p)
is an isomorphism. By induction ???(?) is an isomorphism for m > r+ 1. Hence
???{?) is an isomorphism. By Proposition 18.1 this is identified with CH(<p).
Now apply the Lemma above (and its proof) with ? = ?(?). ?
(d) Tensor products and extra structure.
In this topic we restrict to the case Jk is a field.
Suppose (M,d,3) and (?,?,'?) are filtered differential (graded) modules. The
tensor product is the differential graded module (M,d) <g> (N, d) equipped with
the filtration
FP(M <g> N) = + F{M <g> FjN
i+j=p
(note: the sum on the right is NOT direct). It is straightforward to identify that
if QM or QN is ic-projective then
G{M®N) =GM®GN
as bigraded modules, compatible with the differentials.
Now we observe there are natural identifications
{Er{M®N),dr) s (Er(M),dr)®(Er(N),dr), r > 0 ,
compatible with the isomorphisms Er+i = H(Er,dr). In fact, when r = 0 this
is just the remark above. For r > 1 it follows by an inductive application of the
fact that homology commutes with tensor products (Proposition 3.3).
266 18 Spectral sequences
Example 1 Filtered differential graded algebras.
Suppose (A,d) is a dga equipped with a filtration {Fp} such that Fp is pre-
served by d and satisfies Fp ¦ Fq C Fp+q. Then multiplication
(A,d)®(A,d) —» (A,d)
is filtration preserving and hence determines a morphism of spectral sequences
(Er,dr)®(Er,dr) —>(Er,dr) .
This identifies each (Er,dr) as a differential graded algebra with H(Er) —
Er+\ as graded algebras: we say (Er,dr) is a spectral sequence of graded algebras.
If the spectral sequence is convergent then E^ is a graded algebra. In the case
of a first quadrant filtration the isomorphism E^ — GH{A) is an isomorphism of
algebras. However the graded algebra TotQH(A) may not be isomorphic with
? (A) !
Exercises
1. Let (AV,d) be a Sullivan model. Consider the filtration FP(AV) = A-PV of
example 3 and the associated first quadrant spectral sequence of algebras:
Ep'q = (ApH(V,d0))p+g =» Hp+o{AV,d) .
Prove that if (aV,d) is a 1-connected minimal model then E2 = H(AV,d\).
When (AV,d) is the minimal model of CPn, ? > 3, compute the differentials
and the product in E^·
2. Let (B,d) -» (B ® AV,d) -» (aV,2) be a relative Sullivan model and
consider the spectral sequence of algebras defined in example 2. Prove that
E\'q = (Bp <S> Hq(AV,d),di), and determine di using the short exact sequence
0 -»· Fp+1/Fp+2 -»· Fp/Fp+2 -»· Bp ® AV ->· 0. Prove that if Bl = 0. then
3. Let (AU ® AV, d) be a relative Sullivan model such that dim V < oo and such
that the algebra H(AU,d) is finitely generated. Denote by at- for i = 1.2..... ?
(resp. ??) a linear basis of the space (H+(AU)/H+(aU) ¦ H+(AU))'V" (resp. of
the space V). Prove that if dvi = cq for some cocycle ai in AU representing a·;,
then dim H(AU <g> ??·7, d) < oo.
4. Let F -> X -> F be a fibration as in theorem 15.3. Construct a spectral
sequence (the rational Serre spectral sequence):
P
a) Prove that H(j) : H*(X;Q) -> H*{F;Q) is onto if and only if the ra-
tional Serre spectral sequence collapses at the J52-term and thus H*(X;Q) —
H*(Y;Q) ®ii*(F;Q) as graded vector spaces.
b) Prove that if H*(F; Ik) = Ax with ? of odd degree then H,{X,Ik) is
isomorphic to ??(?, Ik)/(dx) ? sd's XA where A denotes the annihilator of dx in
Graded Differential Algebra 267
5. Show that the filtration of a CW complex X by its skeleton induces a first
quadrant spectral sequence
which collapses at the E-i-term.
6. Suppose that ??2? = 0 unless q = 0 or q = n. Show that E^n — Ker dn+\.
Deduce the short exact sequence
0 _> E&n -» E*>n dnA' ??+?+1'° -> ??+?+?'° -> 0.
7. Let F -4 I -> y be a fibration with 1-connected base Y¦ Suppose further
that H'(Y;Q) = 0 for 1 < i < ? and Hj(F;Q) = 0 for 0 < j < q. Prove that
there is a long exact sequence
0 -» H1 (X; Q) H-J Hl (F: Q) -*· H2 (Y; Q) H^4 H2 (X; Q) -»· ¦ · ·
j.
8. Consider the Serre fibration G/iiT -> 5iiT -4 BG as defined in §15 f, example
1. Deduce from exercise 4b that H*(BK;Q) is a finitely generated H*(BG;Q)-
module via H'(Bj;<Qf).
19 The bar and cobar constructions
In this section the ground ring is an arbitrary commutative ring k. As usual
- <g> — means — <8>/t —.
The bar and cobar constructions are functors
augmented ? co-augmented
differential graded algebras differential graded coalgebras
and
co-augmented ? augmented
differential graded coalgebras differential graded algebras.
They were introduced, respectively, by Eilenberg-MacLane [48] who showed that
B(Z[T]) ~ C, (K(T, 1)) and by Adams [1] who showed that UC»{X) ~ <7,(?,?).
Here we give the constructions, and establish those few properties that will be
essential in the sequel.
We begin with some conventions (cf. §3). The suspension sV of a graded
module is defined by (sV)k = Vk-i and the correspondence is denoted by sv <-* v,
? € V. Similarly {s'1V)k = Vk+i and s~xv <-> v.
Recall that the tensor algebra on a graded module V is the graded algebra
TV = 0 TkV with T°V = Me and TkV = V ® - - - ® V (k times). The multipli-
cation TkV <g> TeV —> Tk+iV is just the tensor product, and TV is augmented
by ? : V -> 0.
The tensor coalgebra on V is defined analogously. It coincides with TV as
a graded module, so to avoid confusion we denote its elements by [v\\ ¦ ¦ ¦ \vk]
instead of by v\ <8> · · · <8> Vk ¦ The diagonal in the tensor coalgebra is defined by
• M = [?>?| · · · \vk] ® 1 + 2_j[vi\ ¦ ¦ ¦ \vi] ® [vi+i\- ¦ ¦ \vk] + 1 ® [wi| · · · \vk].
The tensor coalgebra is augmented by ? and co-augmented by it = T°V. Note
that the diagonal is not compatible with the tensor algebra structure.
Now suppose ? : {A, dA) —> Ik is an augmented dga and denote kere by /.
Definition The bar construction on (A, dA) is the co-augmented differential
graded coalgebra ? A defined as follows:
• As a co-augmented graded coalgebra ? A is the tensor coalgebra T{sl) on
si.
• The differential in ? A is the sum d = d0 + di of the coderivations given by
k
do([sai\---\sak]) = -^(-l)ni [scn\ ¦ ¦ ¦ \sdAa.i\ · · · \sak]
i=l
Graded Differential Algebra 269
and
? dl([sa])=O
) k
di([sai\---\sak]) = ? (-l)ni[«ai| · · · |sui-iui| · · · \sak].
{ i=2
Here nt- = ? deg saj.
j<i
Notice that do reflects the differential d^ in A while d\ is defined using the
multiplication. To see that d2 = 0 note that dg = 0 because d\ = 0, dod\ +dido =
0 because dA is a derivation in A and dj = 0 because A is associative.
We shall adopt the notation ? ? = ? BkA with BkA = Tk(sl). The notation
k
lBA' is an abuse; we should really write B(A,d) or ?(?,?,?), and we will in
fact write B(A, d) when there are several possible differentials in A.
More generally, if (M, d) is a left (A, d^-module (cf. §3) then the bar construc-
tion on {A,dA) with coefficients in (M,d) is the complex B{A;M) = ? A <g> M
with differential d = do + d\ where
k
do[sai\ ¦ ¦ ¦ \sak]m = - ? (-!)"''[s°iI · · · \sdAai\ ¦ ¦ ¦ \sak]m
-(-l)^+i [sai\...\sak}dm
and
k
d\[sa-i| · · ¦ \sak]m = ? (-l)ni[sai| · · · ????-??,? ¦ ¦ ¦ \sak]m
i=2
+ (-l)n*+> [sai| · · · \sak-i]ak · m.
Of course dorn = dm, dim = 0 and di[sa]m = ( —l)dega+1a · m.
We also adopt the convention: Bk{A;M) = BkA ® ?, and elements in BkA
or Bk(A;M) have wordlength k.
Note as well that if we consider ic as an (^4, d^-module via ? then EA, d) =
(B(A;k),d).
Henceforth we shall denote our differential graded algebras by (^4, d) since it
will always be clear from the context whether "d" refers to the differential in .4
or in B(A;M).
There are two situations in which it is easy to compute ? (?(?; ?)). The
first arises when .4 is a tensor algebra. Thus suppose
A = TV and either V = {K-}i>o or {l/i}i>2-
Let dy : V —> V be the linear part of the differential in A, so that d — dv '¦
V —». T^2V, and define
d:sV-^ sV
by dsv = —sdyv.
270 19 The bar and cobar constructions
Now here / = T-^V. Define a morphism of complexes
?: (B(TV,d),d) —> (sV®Jk,d)
by the conditions:
?? = 1, g[sv] = sv, g[s(vi ® · · · ® vi)} = 0, t > 2
Proposition 19.1 With the notation above, ? is a quasi-isomorphism.
proof: Since ? is surjective we need only show ii(ker ?) = 0. Define h :
B(TV) —> ker? by h{\) = 0 and
J [sv\···] —»?, ? G F
^: { v2 ® ¦ ¦ ¦ ® ve)\ ¦ ¦ ¦}, e>2.
A quick calculation shows that hdi +d\h = id in ker ?. Since h raises wordlength
and do preserves it, it follows that (id —hd — dh) increases wordlength in ker ?.
Similarly, id -hd- dh preserves degrees.
Let ? 6 ker ? be a cycle of degree n. Then (id —hd — dh)nz has degree ? and
wordlength at least ? + 1. However, our hypothesis on V implies that elements
in B^n+l(TV) have degree at least ? + 1. Thus (id -hd - dh)nz = 0. Since
id -hd - dh)kz = 0 this gives (id -dh)nz = 0; i.e.
is a boundary. D
The second situation where we can compute is when we take (A, d) as a left
module over itself, via multiplication. This yields the complex
? {A; A) =BA®A.
Note that B(A;A) is a right (A,d)-module via multiplication on the right and
that B(A;M) = ? (?; ?) ®? ?.
Proposition 19.2
(i) The augmentations in ? A and A define a quasi-isomorphism, ? <8> ? :
? (A; A) -% Jfe.
(ii) If k is a field then ? (A; A) is a semifree right (A, d)-module. Thus ?® ?
is an (A, d)-semifree resolution of k.
Graded Differential Algebra 271
proof: (i) Define h : ? (A; A) —> ker(e ? ?) by
[sail ¦ ¦¦\sa.k\a ?—> (-l)e[sa.i\ ¦ ¦ ¦ \sa.k\s(a - ??)]?, k > 1
where e = deg[sax| ¦ · · |sat]a. An easy computation gives id = dh + hd in
ker(c ® ?), so that H(ker(e ? ?)) = 0.
f«j Since ? (A; A) is the increasing union of the submodules B-kA<g>A,
it is sufficient to show that (Lemma 6.3) the quotient modules (B-kA/B<kA) <g>.4
are (A, d)-semifree. These quotients may be identified as (Tksl,do) <8> (-4, d).
Thus the inclusions kerdo® (A,d) c—>(TksI,do)®{A, d) exhibit these quotients
as semifree. ?
The cobar construction is a precise dual analogue of the bar construction.
Suppose (C, d) is a co-augmented differential graded coalgebra with comultipli-
cation ? : C —> C ® C (cf. §3(d)). Let C be the kernel of the augmentation
e:C —»· Jk. Then (cf. §3(d))
Ac - (c ® 1 + 1 ® c) G C ® C , ceC.
Define ? : C —^ C <8 C by Ac = Ac - c ® 1 - 1 ® c. This map is called the
reduced co-multiplication and is also coassociative: (A®id)A — (id ®?)? : C —>
C ® C ® "C.
Definition The cobar construction on (C, d) is the augmented differential graded
algebra QC defined as follows:
• As an augmented graded algebra UC is the tensor algebra T(s~1C) on
The differential in UC is the sum d = do + d\ of the derivations determined
by
do(s~1x) - -s'^x, ? e C
and
dxis-'x) = ^(-l^s^Xi ® s-'yi, xeC,
i
where Ax — Y^Xi®yi.
Exercises
1. Let / : (A,d,e) —> (A',d',e') be a morphism of augmented chain algebras,
then the formula Bf([sai\sa{...\sak}) = [sfal\sfa2\...\sfak], k > 0, defines a
morphism of graded differential coalgebras Bf : ? ? -> ? A'. Prove that Bf is
a quasi-isomorphism if and only if / is a quasi-isomorphism.
272 19 The bar and cobar constructions
2. Let (A,d,e) be a differential graded augmented algebra. Prove that the nat-
ural morphism of differential graded algebras QBA —>· .4 is a quasi-isomorphism.
3. Let (A, d, e) be a 1-connected augmented cochain algebra and denote by B*A
the graded dual of the bar construction ? A. Prove that B*A is a chain algebra.
Prove that if A is of finite type then QA* 9* B*A. Let (A,d) = (AV,d) be a
minimal model and di : V —>· V <8> V be the quadratic part of the differential
d. Prove that V = sH+(B*A) and that di is dual to the multiplication in
H+B*A.
4. Let (A,d,e) and B*A be as in exercise 3 and denote by (/, a) the pairing
B*A <8> ? A -> Ik. Prove that the action defined by / · [sai|sa2|...|safc]a =
, [sai|sa2|...|sat·]) [sai+1|sa2|-.-|safc], k > 0, makes B(A,A) into a right
B*A-module and that this structure is compatible with the right ^4-module
structure. Show that the natural map B*A -> EndA(B(A, A)) is a quasi-
isomorphism.
20 Projective resolutions of graded modules
In this section the ground ring is an arbitrary commutative ring, Jk-
Our objective is to set up the classical homological functors Ext and Tor
for modules over a graded algebra; of course this parallels (and includes) the
standard construction in the non-graded context. Our presentation is terse, and
the reader seeking a more detailed explanation is referred to [118]. The reader
may also wish to review the definitions in §3(a) and §3(b).
Let A be a graded algebra (over ic). A chain complex ({A/*,,}, d) of A-modules
is a sequence
r\ d ? r d f. r d d ¦% r d
0 <— A/o,* <— Mi,* <—¦¦¦<— A/*,, <— ¦ ¦ ¦
in which:
• Each A//.,» is an .4-module, with ?^,? the component of A/*,» of degree
k + i.
• Each d is an ?-linear map of degree —1; i.e.. d : Mkj —> ?/^-?,?.
• dr = 0.
Notice that (cf. §18(a)) (Tot(A/),d) is an (.4,0)-module as defined in §3(c); the
difference is that here we keep track of the bigrading.
A chain map of bidegree (p, q) between two such chain complexes is a family
of ^-linear maps
? : M*,. —> Nk+P,m , k > 0 ,
of degree ? + q, and satisfying dtp = ( — l)p+qtpd.
Note that the homology, H{M*^,d) of a chain complex of ,4-modules is itself
a family {//*,„} of graded ?-modules and that the chain map ? induces -4-linear
maps Hk,» (?) : Hk,, (A/) —> Hk+P,. (N) of degree p + q.
This section is divided into the following topics:
(a) Projective resolutions.
(b) Graded Ext and Tor.
(c) Projective dimension.
(d) Semifree resolutions.
(a) Projective resolutions.
Let A be a graded algebra. An /1-module ? is projective if ? ? Q is .4-free
for some second ,4-module Q (cf. §3(b)). In this case any /1-linear map (of any
degree) ? : ? —> M lifts through any surjective .4-linear map ? : ? —> M.
Indeed, extend ? to ? ? Q by setting ? = 0 in Q, let {vQ} be a basis of ? © Q
and define ? : ? ? Q —> ? by avQ = nQ where nQ G ? satisfies ??? = ava.
Denote A>0 by A+ (cf. §3).
274 20 Projective resolutions of graded modules
Remark 1 If A ~ k ® A+ then any A-projective ? concentrated in degrees
> m, some m G Z, is ?-free.
In fact write ? ? Q = V <g> A for some ?-free module V. Apply — ®.4 k
to obtain (P ®a k) © (Q <8U &) = ^- Thus ? = ? <8u Je is Jc-projective
and splits back into P. This inclusion extends uniquely to an ^4-linear map
? : ? ® .4 —>· P. But clearly ? = ?? ? ? · .4+ = ?? · ? + ? · ?+. Iterating
gives ? = ? ? · ? + ? -(?+ ?+ ). Choosing ? — m + 1 factors A+ we see that
Pn = {?? · A)n\ i-e., ? is surjective. Choose an ^.-linear map ? : ? —> ? ® ?
so ?? = id. Then ??{?) © (? ® A+) = ? ® A and it follows as with ? that r is
surjective. Hence r is an isomorphism, and ? is /1-free.
A projective (free) resolution of an /1-module, M is a chain complex (P, d) =
({Pk,*},d) of projective (free) /i-modules together with a morphism of degree
zero, ? : .Po,* —> M such that
is exact.
Remark 2 Think of M = {MOj,} as a trivial chain complex of .4-modules.
Then a projective (free) resolution is just a quasi-isomorphism ? : {P,d) —>
(M,0) of bidegree @,0).
Remark 3 Suppose ? : (P,d) —> (M,0) is a free resolution. Regard ? as a
quasi-isomorphism (Tot(P),d) -=> (M, 0). Filtering Tot(P) by the submodules
/ k \
Tot I 0 jPj * I then exhibits this as an (.4,0)-semifree resolution of (M, 0).
' /
Analogous to Proposition 6.6(i) we have
Lemma 20.1 Every A-module M has a free resolution.
proof: Choose an .4-linear surjection of degree zero, ? : Po.« —> M, from a
free .4-module POi«. If
<— Po,* <— ¦¦¦ <— Pfc,«
is constructed choose an .4-linear surjection d of bidegree (-1,0) from a free
.4-module Pfc+i,, onto kerd C Pfc,*- D
Next, suppose given the diagram
(N,d)
?
(P,d) . (M,d)
Graded Differential Algebra 275
in which the objects are all chain complexes of -4-modules and ? and ? are
morphisms respectively of bidegrees (p.q) and (??,??).
Analogous to Proposition 6.4(ii) we have
Lemma 20.2 Suppose the A-modules Pfc,« are projective and ? is a surjective
quasi-isomorphism. Put r = (p + q) — (m + ?). Then
(i) There is a morphism ? : (P,d) —> (N,d) of bidegree (p — m,q — n), and
such that ?? = a.
(ii) If ? is a second such morphism then ? — ? = dj + ( — l)rjd, for some
?-linear map 7 : ? —> ker ? of bidegree (p — m + 1, q — 71).
proof: (i) Suppose ? constructed in P;it, i < k. Since Pfc,« is projective there
is an .4-linear map ?1 : Pki. —> ? such that ??' = a. Clearly d?' - {-l)r?d
sends Pk,, into the cycles of ker ?. Since, by hypothesis, ? (ker ?) = 0, it follows
that d : ker ? —> cycles(ker?) is surjectrve. Choose ?" : Pfc-, —> ker ? so that
d?" = d?' - (-l)r?d and set ? = ?' - ?" in Pfc,».
(ii) Note that ? - ? : (P, d) —> (ker?,d). If 7 is constructed in Pi>;
i < k then ?-?-(-\??? sends Pfc,, into the cycles of ker ?. Again, d : ker ? —>
cycles(ker?) is surjective, which permits us to construct 7 : P*^, —> ker ? so that
d-y = ? - ? - (-ly-yd. ' D
(b) Graded Ext and Tor.
Fix a graded algebra, ^4, and -4-modules M and ? (both left, or both right),
and choose a projective resolution ? : (P = {Pfc»},(i) -=> (M,0). Then
HomJ4(Pfci«,iV) is the graded module of .4-linear maps (cf. §3(b)), and
0—>EomA(P0i,,N) ?????(??,?') A--- B0-3)
is the cochain complex of graded modules defined by O(f) = —( — l)de&ffd.
We use the standard convention to raise degrees, and denote the graded module
HornA(Pfc,.,N) by Horn*1*(P,iV):
Eom^(P,N) = Hom,(Pt,t,iV)_(Hi) .
Thus the cochain complex B0.3) has the form (Hom.4 (?, ?),?) with <5 of bidegree
A,0) and its homology is a bigraded A--module, ?*'* (Hom.4(P, ?),?).
- If ?'.: (P',d) -=> (?'/,?) is a second projective resolution then Lemma 20.2(i)
provides us with a morphism ? : (P,d) —> (P',d) such that ?'? = ?, while it
follows from Lemma 20.2(ii) that ? (HomA(?,N)) is independent of the choice
of ?. Reversing the roles of (P, d) and (P', d) yields a morphism ?' : (P', d) —>
(P,d) and Lemma 20.2(ii) implies that ? ?' ~^ idP> while ?'? ~? idP. Thus
? (Hom.4(/3,iV)) and ? (HornaW, ?)) are canonical inverse isomorphisms, and
we use them to identify H'>' (EomA(P,N)) with H*·* (RornA(P',N)). Thus we
may make the
276 20 Projective resolutions of graded modules
Definition For each k > 0, Ext A(M,N) is the graded module
Hk'' (RomA{P,N),o). (Where necessary we write Ext^(A/,iV) = Hk<e; recall
that this is the component of degree k + i.)
Notice that this construction is functorial. Indeed, if ? : M' —> M and
? : ? —> ?' are morphisms of ,4-modules then (Lemma 20.2) ? lifts to a
morphism ? : (P',d) —> (P,d) between projective resolutions for M' and M
and HomA(u,/3) is a morphism of cochain complexes inducing the canonical
linear maps
ExtA{a,?) : ExtkA(M,N) —>ExtkA(M',N') .
In the same way, if L is any ,4-module (left if M is right, right if M is left)
then
0 <— Po,» ®? L i Pi,« 0.4 L i · · · B0.4)
is a chain complex of graded modules (? ®a L = {Pk,, ®a L},d) whose homol-
ogy is a bigraded module independent of the choice of resolution.
Definition For each k > 0, Tor?(M, L) is the graded module Hk,* (P ®a L, d).
(Where necessary we write TorJ??(M, L) = ?^,?\ this is the component of degree
Notice that this construction too is functorial. If ? : M' —> M and 7 : L'
then lift ? to a morphism, a, of projective resolutions and define
We turn now to the elementary properties of these functors. Observe first that
if ? : (?, d) —> (M, 0) is a projective resolution then the surjection ? : Po,* —> M
induces natural isomorphisms
Ext?t (M, N) = YiomA (M, N) and Toro (M, L) = M ®A L . B0.5)
Next, suppose
0 —> M' A M ? M" —> 0
is a short exact sequence of j4~linear maps. (We do not require that ? and ? have
degree zero.) Let ?' : (P',d) -% (M',0) be a projective resolution. It is trivial
to modify the construction in the proof of Lemma 20.1 to obtain a projective
resolution ? : (?, d) -=> (M, 0) of the following form: each Pkt, = P'k ,©P^, each
Pi,» is projective, and the inclusion ? : P' C ? commutes with the differentials
and satisfies ?\ = ?'.
Graded Differential Algebra
277
In this case the quotient (?/?', d) is the chain complex of projective modules
?",. The induced row exact diagram
?
0
(P\d)
(M',0)
(P,d)
(A/, 0)
{P/P',d) —- 0
(A/", 0)
0
exhibits ?" as a projective resolution of M" (pass to the long exact homology
sequences and apply the Five lemma 3.1).
Note that (forgetting differentials) ? = ?' ? ?/?' as families of .4-modules.
Hence if ? is any yl-module,
0 <— EomA(P',N) <— EomA(P,N) <— HomA(P/P',N) <— 0
is a short exact sequence of cochain complexes of graded modules. Passing to
homology gives the long exact sequence
Ext*+1|
ExtkA(M',N) <— ExtkA(M,N) <— ExtA(M",N)
Similarly we obtain the long exact sequence
B0.6)
B0.7)
Finally, if Q is a projective .A-module then Hom>i(Q, —) and Q ®a — preserve
short exact sequences (this is immediate from the definition). From this it follows
that Honru(P, —) and ? ®a — convert short exact sequences
0 —> N' —> ? —> ?" —> 0 and 0 —> V —> L —> L" —> 0
into short exact sequences of complexes, whence the long exact sequences
—> ExtkA{M, N') -^ Ext^(A/, N) —> ????4(?/, ?") ? ????4+x(A/, N')
and
B0.8)
Torj?(M, L)
f,L")
d.
¦k-i(M,L') . B0.9)
Example 1 The bar construction.
Suppose k is a field and .4 -^> k is an augmented algebra, with no differential.
Then the bar construction with coefficients in A (§19) has the form
278 20 Projective resolutions of graded modules
and Proposition 19.2 exhibits this as an .4-free resolution of Ik. But for any left
A-module ? we have B(A; N) = ? (?; ?) ?? ?. It follows that
TorA(lk,N) =H(B(A;N)) .
(c) Projective dimension.
As usual, fix a graded algebra, A. The projective dimension of an /1-module
M, denoted proj dim ? (?), is the least k (or oo) such that M admits a projective
resolution of the form
M <— Po,. <— Pi,. < <— ?*,. <— 0 .
Proposition 20.10 For any A-module, M,
proj dim a (M) = sup I k | Ext* (M, —) is non-zero \ .
proof: Denote the right hand side of the equation by p(M). If M has a projec-
tive resolution of the form above then we can use it to compute Ext4(M, —) and
it follows at once that Ext^(M, — ) = 0, ? > k. Hence p(M) < proj dim^M).
Conversely, suppose p(M) is finite and let ? : ({Pk,*},d) —> (M,0) be any
projective resolution. For simplicity write p = p(M). Let ? C Pp,» be the
submodule of cycles (Z = kerd). Then the differential, d, maps ??+?,« onto Z;
denote this linear map by / : ??+?,« —> ?.
In particular / G ?????+1·*(?, Z) and 5f = fod = d2 = 0. Since ????+1(?, -)
0 by hypothesis, / must satisfy / = god for some g : PPi« —> Z. In other words,
for ? 6 PP-fi,«, dx = f(x) = g(dx). Thus g restricts to the identity in ? and
PPi« = ? ® kerg. In particular kerp is projective and
M <— Po,, 4 <H- Pp_!,» A- kerg <— 0
is a projective resolution, whence proj dim^M) < p(M). ?
(d) Semifree resolutions.
Now let (A, dA)besi differential graded algebra and let m : (P, d) -% (?/, d) be
a semifree resolution of an arbitrary right (A, rf/i)-module, (M,d). As observed
in the Remark at the start of §6(a) (with completely different notation!) we may
use a semifree filtration of (P, d) to write
oo
P = V®A, V = ffi V(k) ,
Graded Differential Algebra 279
where each V(k) is a free graded (Jk—) module and the semifree filtration is given
by P(k) = 0 V(i) ® -4. In particular d : V(k) —> P(k - 1).
i=0
Now m restricts to m@) : V@) ® (A,dA) —> (M,d) . Moreover the filtration
gives rise to a homology spectral sequence (§18) which we denote by (?\d!) .>Q.
Clearly E°k = V(k) ® (.4,d^) and so El = H (E°,d°) = V(k) ® H{A) because
V(k) is .fc-free. Thus we obtain the sequence
H{M) <H{rn{0)) V@) ® H{A) ?- V{\) ® H (A) <
in which all but the first term form a chain complex of H(A)-modu\es.
Definition If this sequence is exact, and hence a free resolution of the H(A)-
module H{M) then m : (P,d) -=» (M,d) is called an Eilenberg-Moore resolution
of (Af,d).
Proposition 20.11 Each (M,d) has an Eilenberg-Moore resolution. More
precisely, any H(A)-free resolution of H(M) appears as the E1-term of some
semifree resolution of (M,d). proof: Fix a free resolution
H(M) A V@) ® H (A) A V(l) ® H (A) 4 .
oo
Set V = 0 V(k) and ? = V <g> A. We define the differential, d and the map m
k=0
in each V(k) by induction on k.
Set d = 0 in V@) and, if {va} is a basis of V@), define m@) : V@) —> M
by requiring m@)va to be a cycle representing gva. Then H(m@)) = ? and so
H(m@)) is surjective.
Now d = id®dA in K@) ® A. Let {f^} be a basis of V{\) and let ?? be a
eycle in V@) ® A such that dvp = [z0]. Then [mz?] = gdv? = 0 G H{M); i.e.,
???? = da:^, some a:^ G ?·/. Extend m and d to A®V{\) by setting dv? = ? ?
and ??? ? = ??.
?
Write ?(?) = 0 V(i) ® ?, ? > 0, and suppose inductively that m and d are
;=o
extended to some P{k), k > 1. Write d = ?] d, with d0 = fd®d^ and d, an
i=0
/1-linear map sending each V(i) to F(?—i)(g).4, i > 1. Thus dxdo + dodx = 0 and
our inductive assumption is that H{d\) = d : V(i) ®H(A) —> V(? — 1) ® H(A).
To extend m and d to V(k+ 1) we consider a do-cycle ? G V(k) ®A such that
d[z] = 0, [2] denoting the class in V(k) ® H(A), and we show that
• There exist w G P(k — 1) and ? G ?/ sue/i that d(z — w) = 0 . ,
and m(z — w) = dx. *·
The construction proceeds via the following steps.
280 20 Projective resolutions of graded modules
Step 1: Since d[z] = 0, dYz = dQz' (z' G V(k-1)®A) and d(z-z') G P{k-2).
Step 2: Suppose for some y' G P(k-l) that d{z-y') = J2ui with Uj € V(i)®A
?
and 1 < ? < k — 2. Then Edu, = 0, whence doue = 0 and d\Ut = —doue-i·
This gives d[u(] = 0. By exactness [u(] = d[u'} for some do-cycle u', and so
ut = d1ul + dou", with u' ?V{?+l)®A and u" G V{?) ® A.
Altogether we have d(z -y' -u' - u") G P{i - 1). Iterating this procedure we
find y G P(k - 1) so that
d(z - y) G V@) ® A .
Step 3: The class [d(z - y)] G V@) ® H(A) may be non zero, but ?[?{? - y)]
is represented by the boundary dm(z - y) and so g[d{z - y)) = 0. By exactness
[d(z-y)] = d[yx] for some <20-cycle yi e V(l) ® A Thus for some y0 G V@) ® .4
we have
d(z - y) = dyi + dy0 .
Step 4·' The cycle ? — y — y\ — yo is mapped by m to a cycle, which is necessarily
of the form m(z0) + dx for some cycle z0 e V@) ® A. Set w = y + yx + y0 + z0.
This completes the proof of B0.12). Now let {^a} be a basis of V(k + 1),
write dv\ = [z\] and note that d[z\] = d2v\ = 0. By B0.12) there are elements
w\ € P(k — 1) and x\ G M such that d(z\ — u>\) = 0 and m(z\ — w\) — dx\.
Extend d and mtoV{k+\)®Aby setting dv\ = z\ — W\ and mv\ = x\.
This completes the construction of d and ??. Clearly m is a morphism of
(^4,dJ4)-modules, (P, d) is semifree and the u^-term of the spectral sequence is
as desired. Finally, since H(m@)) is surjective, H(m) certainly is.
To show H(m) is injective let ? be a class represented by a cycle ? G P(k).
Put ? = ???, Zi G V(i) ® A. Then dozk = 0 and d[zk) = 0. By exactness
[zk] = d[w'] and so Zk = diw' + dQw" for some do-cycle w' G V(k + 1) ® A, and
some w" G V(A;) ® .4. It follows that ? - d(w' + w") G P(k - 1).
In summary, any class ? in H(P) is represented by a cycle z0 G V@) ® A. If
H(m)a = 0 then ?[?0] = 0, [?0] = 9[2{] and z0 = dB{ + ?'?); i.e. ? = 0. D
Example 1 The Eilenberg-Moore spectral sequence.
Suppose (M, d) is a right (^4, dA)-modu\e with a semifree resolution (P, d) -=>
(M, d) as constructed in Proposition 20.11. If (iV, d) is any left (yl,dj4)~module
then the filtration {P(k)} defines the filtration
P@) ®A ? C P(l) ®A ? C · · · C P{k) ®A ? C · · ·
of ? ®A N.
This leads to the (fundamental) Eilenberg-Moore spectral sequence, whose E1-
term is just {{V(k) ® H(A)},d) ®h(a) H(N). The left hand tensorand is an
Graded Differential Algebra 281
i/(,4)-free resolution of H(M). Thus the I?2-term of the Eilenberg-Moore spec-
tral sequence is given by
El. = Torf(A) (H(M),H(N)) .
Under simple hypotheses the spectral sequence converges to H(P<S>a N). As
shown in §6, this homology is a functor, independent of the choice of semifree
resolution. It is called the differential tor, Diff Tor'1 (A/, N) and so the Eilenberg-
Moore spectral sequence converges (usually) from
????{?^(?(?),?(?)) => Diff ????(?,?) .
Exercises
1. Let (?, ?) be an augmented graded algebra and A =ker ?. Using §20 b,
example 1, prove that Torf (Ik, Ik) = A/AA.
2. Let (A, e) be a graded augmented algebra. Compute the projective dimension
of the trivial yl-module Ik when a) A = T(V), b) A = AF,dimF < oo. c)
A = Ik[x]/(xn).
3. Let F -» X -» Y be a fibration with Y 1-connected and let M be a semifree
C*(F; .^-resolution of C*{X;Ik). Prove that C*(P(Y,yo);Ik)) ®c-(Y;ik) M is
a resolution of C*(F;Ik). Deduce the Eilenberg-Moore spectral sequence of a
fibration
4. Let F -> X -> Y be a fibration with 1-connected base and let Ik be a field of
characteristic zero. Assuming that the Serre spectral sequence of the fibration
collapses at the -Eo-term, prove that the graded algebra H*(F,Ik) is isomorphic
to the quotient algebra Ik ®H-(Y-,ik) H*(X, Ik).
5. Let ? —» X be a G-fibration as in theorem 8.3. Using the spectral sequence
associated to a semifree resolution construct a spectral sequence
TorH-{G;rk)(Ik,Hm(P,Ik)) =» H*{X,Ik).
6. Express Theorem 7.5 in terms of differential Tor.
7. Let X = K(G, 1) with universal cover X. Deduce from §8-exercise 1 that
H*(X;Ik) 9i TorA'(G](ifc,ifc) and that H*(X,Ik) 2i Ext?[?](Ik, Ik). As an appli-
cation, compute Ext#.[G] (Ik, Ik) when G is a free group.
8. a) Let ? and F be Z-modules. Prove that Tor^.2(E, F) = 0.
b) Let M and ? be chain complexes over Z. Deduce the following short exact
sequences from the Eilenberg-Moore spectral sequence:
®H.(N))n -> Hn(M®N) -> ep+9=n-iTorf(#p(M),ii?(iV)) _> 0.
282 20 Projective resolutions of grad
c) Let X and Y be topological spaces. Prove the existence of
Kunneth exact sequence:
0 -> (H*(X) ? H.{Y))n -> Hn{X ? ?) ¦
Part IV
Lie Models
21 Graded (differential) Lie algebras and Hopf
algebras
The ground ring in this section is a field k of characteristic zero. S^ denotes
the permutation group on k symbols.
This section introduces graded Lie algebras together with associated construc-
tions such as the universal enveloping algebra. The main example for us is the
rational homotopy Lie algebra of a topological space, defined in (c), whose uni-
versal enveloping algebra is the loop space homology algebra.
We begin with the
Definition A graded Lie algebra, L, is a graded vector space L = {L,}ieZ
together with a linear map of degree zero, L® L —> L, ? ®y ·-> [x,y] satisfying
(i) [x,y] = -(-l)dezxdezy{y,x). (antisymmetry)
(ii) [x,b,2]] = [[x,y],z] + (-l)dezxdezy{y,[x,z]]. (Jacobi identity)
The product [ , ] is called the Lie bracket.
A morphism of graded Lie algebras is a Lie bracket-preserving linear map of
degree zero.
If ? and F are graded subspaces of L then [E, F] denotes the graded subspace
of linear combinations of elements of the form [x,y], ? 6 ?, y 6 F. In particular a
sub Lie algebra (resp. an ideal) ? C L is a graded subspace such that [E, E] c ?
(resp., [L,E] C E). In either case the restriction of the bracket makes ? into a
graded Lie algebra. If ? is an ideal then there is a unique graded Lie algebra
structure in L/E for which the quotient map L —> L/E is a morphism of graded
Lie algebras. Given a subset 5 C L the sub Lie algebra (or ideal) generated by
S is the intersection of all the sub Lie algebras (or ideals) containing 5.
The subspace [L, L] is an ideal, called the derived sub Lie algebra, and L is
abelian if [L, L) — 0; i.e. if [x,y] — 0 for all x,y 6 L.
Example 1 Graded algebras.
Recall that graded algebras A are associative by definition (§3(b)). A Lie
bracket (called the commutator) is defined in A by
[x,y] =xy-{-l)dezxdezyyx
with the Jacobi identity following from the associath'ity. Note that this Lie
algebra is abelian if and only if A is commutative. ?
Let L be a graded Lie algebra. A (left) L-module is a graded vector space V
together with a degree zero linear map L<g>V —> V, ? <8> ? >->¦ ? · ?, such that
[x,y).v = x.(y >?) - (-l)de*x de*yy -(? >?) .
A right L-module is a graded \'ector space V together with a degree zero linear
<dx^V'X such that v[x,y] = (v<x)-y- (_l)uegi teiii(v.y).x. ???
284 21 Graded (differential) Lie algebras and Hopf algebras
is a left L-module the induced linear map ? : L —> Hom(V, V) is a morphism
of graded Lie algebras, where Hom(V", V) is given the commutator Lie bracket of
Example 1. This is called a left representation of L in V.
A morphism of L-modules a : V —> W is a linear map of degree zero such that
a(x ·?) — ? · ??, ? G L, ? G V. Submodules and quotient modules are defined
in the obvious way and the tensor product of two L-modules is the L-module
Vr <g> W given by
x-{v®w) = x-v®w + (-l)degxdeguv®x-u), ? € L, ? 6 V, w 6 IV.
Note that there is no tensor product analogue for modules over an associative
algebra.
Example 2 The adjoint representation.
If L is a graded Lie algebra then a representation ad : L —> Hom(L, L) is
defined by
(adx)(y) = [x,y], x,y 6 L.
This is called the adjoint representation, and (by the Jacobi identity) makes L
into an L-module. The submodules are precisely the ideals in L. D
Example 3 Derivations.
Suppose first that A is a graded algebra. The graded space Der A of deriva-
tions of A (§3(b)) is a graded Lie algebra under the commutator [a, ?] = a? —
(_l)dega deg??a_ jn other words, Der .4 is a sub Lie algebra of Hom(yl, A) with
the Lie bracket of Example 1.
Now suppose L is a graded Lie algebra. A derivation of L of degree & is a
linear map 9 : L —» L of degree k such that ?[?, y] = [??, y) + (-l)kde^x[x, 9y].
The derivations of L form a graded sub Lie algebra DerL C Hom(L, L), again
with respect to the commutator.
Note that the Jacobi identity states precisely that each ad a: is a derivation of
L. Thus ad : L —» Der L. D
Example 4 Products.
The product of two graded Lie algebras ? and L is the direct sum, ? © L,
with Lie bracket
f
In particular for ? G ?, y G L we have [?, y) = 0 in ? ? L.
Example 5 The tensor product, A ® L.
Suppose L is a graded Lie algebra and .4 is a commutative graded algebra.
Then A <g> L is a graded Lie algebra with Lie bracket
[o ® X, b ® y] = (-l)des* des*ab ® [z, y]. D
Lie Models 285
The rest of this section is organized into the following topics:
(a) Universal enveloping algebras.
(b) Graded Hopf algebras.
(c) Free graded Lie algebras.
(d) The homotopy Lie algebra of a topological space.
(e) The homotopy Lie algebra of a minimal Sullivan algebra.
(f) Differential graded Lie algebras and differential graded Hopf algebras.
(a) Universal enveloping algebras.
Let L be a graded Lie algebra and TL the tensor algebra on the graded vector
space, L. Let / be the ideal in the (associative) graded algebra TL generated by
the elements of the form x®y-(-l)dezx dezyy®x-[x,y\, x,y e L. The graded
algebra TL/I is called the universal enveloping algebra of L and is denoted by
UL.
Observe that the inclusion L —> TL gives a linear map ? : L —> UL and that ?
is a morphism of Lie algebras with respect to the commutator in UL. Conversely,
let a : L —> A be any linear map into a graded algebra which is a morphism
of Lie algebras with respect to the commutator in A, then the extension of a to
an algebra morphism TL —> A annihilates / and so factors to yield a unique
morphism of graded algebras
? : UL —> A
such that ?? = a.
In particular a left representation a of L in V determines an algebra morphism
? : UL —> Hom(V, V) such that ?? = a. This identifies left L-modules with left
modules (in the classical sense) over the universal enveloping algebra. Similarly
right L-modules are just right UL-modules.
In the same way if ? : ? —> L is a morphism of graded Lie algebras then the
composite ? -^> L -^» UL determines a morphism of graded algebras
??-.UE —> UL
such that Uip ? ? = ?? ?.
Example 1 Abelian Lie algebras.
If L is abelian then UL = TL/(x®y- (-l)desx dee»y ®x); i.e., UL is the free
commutative graded algebra, AL, defined in Example 6 of §3(b). D
286 21 Graded (differential) Lie algebras and Hopf algebras
In general the algebra UL is more complex than AL. Nonetheless a funda-
mental theorem asserts that UL and AL are isomorphic as graded vector spaces.
The first form of the theorem is by an explicit comparison of bases. For this
we fix a well ordered basis of L : {xa}aej- Define an admissible sequence M
of length k to be a finite (or empty) sequence of indices ai,...,ctk such that
&i < a2 < " · " < ak and such that ??· occurs with multiplicity one if xai has
odd degree. Define the corresponding admissible ?-monomial to be the element
XjX} = ??? ? · · · ? xak G AL (or ?? = 1). Then the admissible ?-monomials are
a basis of AL.
Next define the admissible [/-monomials to be the elements u<p = 1 and
um = {????)¦¦¦ (ixak.) <? UL.
Theorem 21.1 (Poincare-Birkoff-Witt) Let L be a graded Lie algebra. Then
(i) The admissible U-monomials are a basis of UL.
(ii) In particular, the linear map ? : L —> UL is an inclusion and extends to
an isomorphism of graded vector spaces AL -^ UL.
proof: [139] If M = ??,..., ?* is admissible then so is ? = ?2,..., ak and we
write M = ???. We show first that an L-module structure in AL is defined by
the conditions xa · ?? = xa and, if ? = ???,
{xaM if a < ?? or a — ai and deg?a is even.
\{xa,Xa\-XN if ? = ?? and degza is odd.
[??,??,]·** + (-l)dee*edegIei*a1 -Xa'XN if » > »1 ·
Indeed suppose by (transfinite) induction that xa> · xm1 is defined if
length M' < length M or if length M' = length M and a' < a. Assume further
that xa' ·??· is a linear combination of monomials of length < length M' + 1.
Then the right hand side of the formula above is defined, and is a linear combi-
nation of monomials of length < length M + 1. By induction xa :Xm is defined
for all a and M.
To show that this makes AL into an L-module we write
(?,?,?) = [x«,X?\-xM-x«-X?>XM+{-l)de%Xa deeX0X?'X*'XM
and then verify that (?, ?, A4) = 0 for all ?, ? and M. For this we may clearly
suppose ? < a and induct, as before, on length M and on ?.
It is immediate from the defining formula that {?, ?,?) = 0. Now suppose
by induction that (?',?',?1) = 0 if length M' < length M or if length M' =
length M and ?' < ?. Write ? = ???, so that xm = Xai · xn- We proceed in
four steps.
(i) If ? < »? or ? = ai and degz/? is even then ??'?? = ??? and the
defining formula gives (?, ?, ?) = 0.
Lie Models 287
(ii) If ?? — ? = a and if deg xp is odd then by our inductive hypothesis on
length M,
(?,?,?) = [??,??] ·?? ·?? - 2?? -(?? -?? ·??)
= [??,??]·?? ·?? - ??'[??,??]·??
= [[??,??],??]·??.
The Jacobi identity implies 3[[??,??],??] = 0, whence (?,?,?) = 0.
(Hi) If ?; = ? < a then xa · ? ? is a linear combinations of monomials xm> with
either length M' < length M or else ?' = ?iN' with ?? > a. Thus (i) and the
inductive hypothesis on length give ? ? ·??·?? ·?? = -y [? ?, ??] ·?? ·?^. Now
a simple calculation using the Jacobi identity and induction on length yields
(?, ?,?) = 0.
(iv) Suppose ? > ai. Use induction on length and the Jacobi identity to verify
that (?, ?, M) is a linear combination of expressions of the form (?, ai, M') and
(?, ??, M') with length M' < length M. Now the inductive hypothesis implies
{?, ?,?) =0.
This completes the proof that the defining formula above makes AL into an L-
module. The action of L extends to an algebra morphism UL —> Hom(AL,AL)
and the linear map UL —> AL defined by a ·-*· a · 1 sends um to xm. Since the
?? are a basis for AL the um are linearly independent.
On the other hand, UL is generated as an algebra by t(L) and hence is linearly
spanned by monomials of the form i(xai) t(^at) where no restriction is
placed on the order of the ??·. However, by construction, we have t(x)t(y) —
(-l)desx dezyi{y)L{x) = t[x,y] and i(xJ - \l([x,x\) if \x\ is odd. It follows that
any monomial can be written as a linear combination of an admissible monomial
um and monomials of shorter length. Induction on monomial length now shows
that every element in UL is a linear combination of the um; i.e. the um are a
basis for UL. ?
Henceforth we shall identify L with its image in UL and drop the notation ? '.
If ? is a permutation of k letters and if ??,..., Xk are elements in a graded
vector space V then in AkV we have ?? ? · · · ? xk = ???^ ? · · · ? ??(^, where
? = ±1 and depends only on ? and the degrees of the X{. Where the context
is clear we abuse notation and denote this sign simply by ??. Recall that 5/t
denotes the permutation group on k letters.
The following is a useful variant of the Poincare-Birkoff-Witt Theorem:
Proposition 21.2 If L is any graded Lie algebra then a natural linear isomor-
phism of graded vector spaces,
7 : AL ? UL
is given by 7 (?? ? · · · ? xk) = ^ ? ????{\) ' ' · Xa(k) ·
S
288 21 Graded (differential) Lie algebras and Hopf algebras
proof: We adopt the notation of Theorem 21.1 and its proof. Let UL(k) denote
the subspace spanned by monomials in L of length < k. The last part of the
proof of 21.1 establishes in fact that the admissible monomials of length < k are
a basis of UL(k). Moreover, if xai ¦ ¦ -xak is an admissible monomial in UL(k)
and if ? is any permutation then xai ¦ ¦ ¦ xah — ???????) ¦ ¦ ¦ ???{1:) ? UL(k — 1).
Hence for any admissible sequence M of length k, j{xm) — u-\j G UL(k — 1).
Since the um with M of length k represent a basis of UL(k)/UL(k — 1) while
the xm are a basis of AkL, it follows that 7 is an isomorphism. D
Corollary If ? Q L is a sub Lie algebra of a graded Lie algebra L then UL is
a free left (or right) UE-module.
proof: Write L = E@F (as graded vector spaces) and identify AL = Ai?® AF.
Define 7' : AE <g> AF —>· UL by 7'(? ® b) = 7@) · 7F). The same calculation as
in the Proposition shows that 7' — 7 : AkL —> UL(k — 1). Hence 7' induces iso-
morphisms AkL JZ^ UL(k)/UL(k— 1), which implies that 7' is an isomorphism.
Since 7(A?) = UE C UL it follows that multiplication in UL defines an
isomorphism UE®^(AF) -^> UL. This exhibits UL as the free left C/fJ-module
on 7(Ai*1). The same argument shows it is a free right U^-module. D
(b) Graded Hopf algebras.
A graded Hopf algebra is a graded vector space G which is simultaneously a
graded algebra and (§3(d)) a graded coalgebra (with comultiplication ? : G —>
G®G and augmentation ? : G —> Je), such that both ? and ? are morphisms of
graded algebras. Note that the multiplication in G is automatically a morphism
of coalgebras and that 1 6 Go provides the coaugmentation. The Hopf algebra
is cocommutative if ?? = ? where r(a <g> b) — (—l)des° deg&e 0 a. Morphisms
of graded Hopf algebras are linear maps of degree zero that preserve all the
additional structure.
Suppose G is a Hopf algebra and recall (§3(d)) that an element a: 6 G is
primitive if ?? = x®l + l®x. If ? and y are primitive then so is the commutator
bracket [x,y]:
A([x,y]) = A{xy-(-l)dezxdezyyx)
= i\xi\y - (-l)de8* de%yAyAx
= [x ® 1 + 1 <8> x,y <8> 1 + 1 <8> y]
= [x, y] <g> 1 + 1 ® [x, y) .
Thus the space P*(G) of primitive elements is a graded Lie algebra with respect
to the commutator bracket.
Conversely, if L is a graded Lie algebra then UL is naturally endowed with
the structure of a cocommutative graded Hopf algebra. To define the diagonal
we recall first that if ? and L are two graded Lie algebras then elements of
? commute with those of L in ? ? L (Example 4). It follows that UE and
UL commute inside U(E © L) and so the inclusions extend to morphism of
Lie Models 289
graded algebras UE ®UL —» U(E ? L). On the other hand the Lie morphism
? @ L —-> [/?¦ <g> UL given by (z, y) >-> ? <g> 1 + 1 <g> y extends to an algebra
morphism U(E ? L) —> UE <g> UL. Since these two morphisms reduce to the
identity in ? and L they are inverse to each other: U(E ®L) = UE <g> f/L as
graded algebras.
When ? = L the diagonal ? : L —> L ? L, ? : ? ?-*· (?, ?) extends to
C/i : f/L —>· f/(L ? L), which we may now write as
?-.UL—iUL® UL.
Similarly, ? : UL —> k is obtained from the trivial Lie morphism L —> 0. These
are both algebra morphisms by construction. From (? ? ??)? = (id ? ?)? we
deduce (? <g> id)?. = (id <g> ?)?. The identity (? <g> ??)? = id = (??®?)? is even
easier. Thus (UL, ?. ?) is a graded Hopf algebra. To see that it is cocommutative
let ? : L ? L —> L ? L be the involution (x,y) *-l· (y, x). Then ?? = ? so that
(?/?)? = ?. But a trivial check shows that (Ua)(a <g> b) = (-l)desQ deebb <g> a.
Note also that the isomorphism 7 of Proposition 21.2 is an isomorphism of
graded coalgebras.
Proposition 21.3 The inclusion L —> UL is an isomorphism of L onto the
graded Lie algebra of primitive elements in UL.
proof: It is immediate from the definition of UL that the inclusion is a mor-
phism of Lie algebras. To see that it is an isomorphism onto P*(UL) define a
Hopf algebra structure in AL with diagonal ?? the unique algebra morphism
given by ?? (?) = x <8> 1 + 1 <8> ?, x G L. Since ? is an algebra morphism a
very short computation shows that the linear isomorphism 7 : AL —> UL of
Proposition 21.2 is an isomorphism of coalgebras. Thus it is sufficient to prove
that L is the primitive subspace of AL. Fix a basis {xa} of L. Since ?? is an
algebra morphism, ?? (?*1, ? · · · ? x^r) can be expanded easily. In particular
it contains a term of the form k\xla <g> (ij'~' ? · · · ? ?^.) and this term cannot
appear in the diagonal of any other monomial in the {xa}- Thus a linear com-
bination of monomials is primitive if and only if they all have length 1; i.e., all
primitive elements in AL are in L. ?
(c) Free graded Lie algebras.
Recall that TV denotes the tensor algebra on a graded vector space V. This
is a graded Lie algebra with the commutator bracket. The sub Lie algebra
generated by V is called the free graded Lie algebra on V and is denoted by Ly ·
This is justified by the appropriate universal property. A linear map V —> L
of degree zero, L a graded Lie algebra, extends uniquely to an algebra morphism
TV —> UL. Now L is a sub Lie algebra of UL (Theorem 21.1 (ii)) and so this
extension restricts to a map hv —> L which is necessarily a morphism of graded
Lie algebras.
Note as well that the inclusion Ly —> TV extends to an algebra morphism
ULy —> TV. On the other hand, the inclusions V c—> Ly c—>· C/Ly extend to
290 21 Graded (differential) Lie algebras and Hopf algebras
a morphism TV —> ULv ¦ Since V generates Ly as a Lie algebra it generates
ULv as an algebra. Since these two morphisms reduce to the identity in V they
are inverse isomorphisms:
TV=ULv ¦
Now as usual we say elements in TkV have wordlength k. Analogously, we
say that an element in Ly has bracket length k if it is a linear combination of
elements of the form [??,..., [vk-i, ?*] · · · ].
Since Ly is generated by V it is the sum of its subspaces of bracket length k.
Since these spaces are contained in TkV we may deduce that
• Ly = ? (Ly DTkV).
it>l
• ? G Ly has bracket length k if and only if ? G Ly ? TkV.
Now let L be any graded Lie algebra and choose any graded subspace V C L
such that L = V ©[L,L]. Extend the inclusion of V to a morphism
? : Ly —> L
of graded Lie algebras.
Proposition 21.4 If L = {L{}i;>1 then ? is surjective. Moreover the following
three conditions are equivalent:
(i) ? is an isomorphism,
(ii) L is free.
(Hi) projdinn/ii*) = 1 (cf. \20(c)).
proof: Put ? = a(Ly); it is the sub Lie algebra generated by V. Clearly
? + [L, L] = L. Since [E, E] C E, substitution gives ? + [L, [L, L]] = L.
Iterating this process gives
? + [L,[L,...{L,L]...,]J = L
for any k. Since L = {Li}i:>1 any obvious degree argument gives ? = L.
It remains to prove the equivalence of (i), (ii) and (iii). Clearly (i) =>¦ (ii).
If (ii) holds then write L = L\v and UL = TW. Let sW be the suspension of
W : (sW)i — Wi-i. Then the free resolution
0 <— Jfc <— TW ^- sW ® TW +— 0 ,
Lie Models 291
d(sw®a) =w<g>a, shows that proj dirndl;) = 1. To show (iii) =?- (i), suppose
proj dime//,($;) = 1. Because L = {-?'i}i>1, k has a free resolution of the form
Of- k^-UL^- Phm<
with Pi,» = {Pi,i}i>0- Now the proof of Proposition 20.10 provides a projective
resolution of the form
with ? C Pi,». Since ? is projective it has the form ? = W ® UL, by the
Remark at the start of 20(a). Thus d embeds W (with a shift of degrees) as a
graded subspace W C UL and it is a simple exercise to see that UL = TW.
Next observe that
{UL)+ = V® {UL)+ -{UL)+ .
In fact since ? is surjective it follows that (UL)+ = V + (UL)+ -(UL)+. On the
other hand, denote the abelian Lie algebra L/[L,L] simply by F. Its universal
enveloping algebra is just AF and the composite map UL —> AF —> AF/A-2F
kills (UL) + -{UL)+ and sends V isomorphically to F. Hence Vn(UL)+ -{UL)+ =
0.
Now filter by the wordlength in TW to see that for any subspace V com-
plementing (UL)+ -(UL)+ in (UL)+ we have UL = TV. In particular we may
choose V = V. Then the identification UL = f/Ly is an isomorphism of Hopf
algebras and hence restricts to an isomorphism L = Ly of primitive Lie algebras
, which is inverse to ?. ?
Corollary IfV — {Vi}i>1 then any sub Lie algebra L C Ly is free.
proof: As noted in the proof of (ii) =$¦ (iii) in the Proposition, k has a C/Ly-
free resolution of length 2. Since C/Ly is a free C/L-module, (Corollary to Propo-
sition 21.2) this is a free C/L-resolution and proj dime//,($;) = 1. ?
Example Free products.
Suppose {L(a)}acj is a family of graded Lie algebras. The free product.
U L(a), is the graded Lie algebra L defined as follows: write V = ®L(a) and
a a
denote by ia: L(a) —> V the inclusion. Let / C Ly be the ideal generated by
the elements ia[x,y] — [iax,iay], x,y € L(a), a G J. Then \J L(a) = Ly//.
a
Note that ia induces a morphism ja : L(a) —> \J L(a) of graded Lie al-
a
gebras, and that any family of graded Lie algebra morphisms ipa : L(a) —> ?
determine a unique morphism ? : \J L(a) —> ? such that ?? = ? oja, a G J.
a
In particular there is a morphism ?? : [] L(u) —> L(a) such that gaja = id
a
a"d Q?ja = 0. ? ? 0. Thus each ja is an inclusion.
292 21 Graded (differential) Lie algebras and Hopf algebras
Next suppose A(a) = Jfc © A(a) are graded associative algebras, with A(a)
— ( ?
an ideal. We define the free product, ]J A(a), and show that U I \J L(a) I =
a \ a J
U UL(a). Indeed, let J be the set of all finite sequences a\,..., aq of index
?
elements such that aj ? chj+i. Set
with the product of ?,? ® · · · ® aq ? -A(a:i) <g> · · · <g> --4(a?) and ?>i <g> · · · <g> ?>r 6
A(a[) ® · · · <g> A(a'r) given by ?? <g> · · · <g> ???>? <g> 60 <8> · · · <8> &r if »? = <*? and by
ai ;8> · · · ® aq ® h <g> · · · <g> ?>r otherwise. Clearly morphisms ·?? : A(a) —> ?
of graded associative algebras extend uniquely to a morphism JJ A(a) —> B.
a
Use this, and the analogous universal property for ?J L(a) to construct inverse
?
isomorphisms between U ]\ L(a) and \J UL(a).
a a
Finally, suppose A = h®A and ? = ic©? are associative graded algebras and
that F» —> h and Qr —> h are respectively an ?-free and a Z?-free resolution
beginning with the augmentations Pq = A —> k and Q\ — ? —> Jk. Note that
AUB is ^4-free on the direct sum of the subspaces B®A®··· and h. It follows
that _
P>\ ®a (? ? ?) -=> A ®A {A U B)
is an A II 5-free resolution. Since A II 5 = A~ ®A (A II5) ? ? ®B {A II B) we
obtain an yl II Z?-free resolution of k of the form
[?>? ®? (^4 U ?) ? Q>i ®b (A U B)} —> ? U ? —> Jfc .
This yields the formulae
Tori*UB(Jfc1 -) = Torf (k, -) ? Torf (Jfc, -) , i > 1
and
Ext^UB(i:,-) = Ext^(l;, -) ® ExtB(J:,-) , i > 1 .
These apply, in particular, to case that A and 5 are universal enveloping alge-
bras.
(d) The homotopy Lie algebra of a topological space.
Let A" be a simply connected topological space. In §16 we defined the loop
space homology algebra, Ht(UX:k). Moreover, the Alexander-Whitney diago-
nal
H(&) : Hr(flX;k) —> Hr(ClX;k) ® Hr(flX; k)
is a morphism of graded algebras, as was observed in Lemma 16.3. The map
ClX —> pt is trivially product preserving and so the canonical augmentation
Lie Models 293
? : Hr(UX;k) —> k is also a morphism of graded algebras. In other words, the
Alexander-Whitney diagonal and the canonical augmentation make Hr(QX;Jk)
into a graded Hopf algebra.
On the other hand, recall that since PX is contractible, the connecting ho-
momorphism for the path space fibration is an isomorphism, dr : ?» (?) -^>
?,_?(?,?). Thus we may transfer the Whitehead product [,}w ???,(?) (§13(e))
to a bracket [ , ] in ?, (??) by setting
[?,?] = (-l)de*a+1dr {[d:la,d:l?)w) , ?,? G ??,(??) .
Theorem 21.5 (Milnor-Moore) [127] If X is a simply connected topological
space then
(i) The bracket above makes ?, (??) <8> Se into a graded Lie algebra (to be
denoted by Lx).
(ii) The Hurewicz homomorphism for ?? is an isomorphism of Lx onto the
Lie algebra P,(flX;Jk) of primitive elements in H*(QX;Se).
(iii) The Hurewicz homomorphism extends to an isomorphism of graded Hopf
algebras,
ULx -=>
proof: Consider first the case that X has rational homology of finite type. The
Cartan-Serre Theorem 16.10 asserts that hur : ?,(??'") <g> Se -^ Pr(UX:Se) is a
linear isomorphism. Hence a unique Lie structure is induced in ?,(??) (8>ic such
that hur is an isomorphism of Lie algebras. Proposition 16.11 asserts precisely
that for ?,? G ?»(??),
[hura,hur?] = (_i)^ga+i hurdr ([?^?,?^?]^ .
Thus the induced Lie structure in ?, (??") ® Se is given by the required bracket.
This proves (i) and (ii). For assertion (iii) note that, by the definition of
universal enveloping algebras, hur extends uniquely to a morphism of graded
algebras ? : ULx —> ?*(??; Je). Theorem 16.13 states precisely that this is
an isomorphism. Since L\ is primitive in ULx (Proposition 21.3) the algebra
morphisms (? ® ?)? and ?(?)? agree in Lx. But Lx generates the algebra
ULx. It follows that (? ® ?)? = ?(?)?; i.e., ? is an isomorphism of Hopf
algebras.
Now consider the general case. A weak homotopy equivalence X' —> X
from a CW complex X' (Theorem 1.4) will induce a weak homotopy equivalence
??"' —> ?? (long· exact homotopy sequence) and hence a homology isomor-
phism H»(QX';Je) -=> H*{UX\Se) (Theorem 4.15). Thus, given the proof of
Theorem 1.4, we may suppose X is a CW complex with a single 0-cell and
294 21 Graded (differential) Lie algebras and Hopf algebras
no 1-cells. Then any singular chain c G C*(X;k) and any continuous map
/ : 5" —> A' will satisfy c € C*(Y;k) and f(Sn) C Y for some finite sub-
complex Y C X. If / : ? —> ?? is a continuous map from a compact set
then all the loops f(y), y G ? have length bounded above by some I. Moreover
{/(?/) @ j 0 < i < ^, y G iv} is a compact subset of X and hence contained in
some finite complex Y. Thus any singular chain c G C, (??; le) and any contin-
uous map f : Sn —> V.X will satisfy c G C*(UY;]k) and f(Sn) C QY for some
finite subcomplex Y C X-
Since the theorem has been proved for finite subcomplexes Y C A it follows
now in general. D
Definition The graded Lie algebra Lx = (?,(???) ® ic, [ , ]) is called the
homotopy Lie algebra of X with coefficients in Ik. When h = Q it is called the
rational homotopy Lie algebra of X.
Observe that all the constructions above are functorial: if / : X —> Y is
a continuous map between spaces satisfying the hypotheses of Theorem 21.5
then ??,(?/) <S> 1; is a morphism of graded Lie algebras and hur is a natural
isomorphism.
(e) The homotopy Lie algebra of a minimal Sullivan algebra.
Let (AV, d) be a minimal Sullivan algebra, as defined in §12. Thus the differ-
ential may be written as an infinite sum d = d\ + g?2 + " ¦ of derivations, with
dk raising wordlength by k. As observed in §13(e), (AV,d\) is itself a minimal
Sullivan algebra.
Define a graded vector space L by requiring that
sL = Hom(V, A) ,
where as usual the suspension sL is defined by (sL)k = Lk-\- Thus a pairing
( ; ) : V x sL —> A is defined by (v;sx) = (-l)degusx(u). Extend this to
(k + 1)-linear maps
AkV ? sLx ¦¦¦ ? sL —» A
by setting
(Vi A ¦¦¦ AVk;SXk,-·· ,SXl) = V" ??(??(?)-,8??) ¦ ¦ ¦ (va(k)-,SXk),
where as usual Sk is the permutation group on k symbols and ??(?) ?- - -???^) =
Definition A pair of dual bases for V and for L consists of a basis (vi) for V
and a basis (xj) for L such that
1 if i = j
0 otherwise.
Lie Models 295
We observe now that L inherits a Lie bracket [ , ] from d\. Indeed, a bilinear
map [ , ] : L ? L —> L is uniquely determined by the formula
(v;s[x,y]) = (-l)de^+1{dlV;sx,sy) , x,yeL,veV.
The relation ? ? w = (-l)deeu aegww ? ? ieacjs at once to
[x,i/] = -(-l)degxdegl'b,x],
and an easy computation gives
(d?u; sx, sy,sz) = (-l)d**v(v,s[x, [y, z]] - s[[x,y],z] - (-I)«1*«» de*vs[y, [x, z]]).
Thus the Jacobi identity is implied by (indeed is equivalent to) the relation
Definition The Lie algebra L is called the homotopy Lie algebra of the Sullivan
algebra (AV,d).
This construction is functorial. If ? : (AV, d) —> (AW, d) is a morphism of
minimal Sullivan algebras then recall (§12(b)) that Q(ip) : V —> W is the linear
part of ? : ?? — Q(tp)v € A-2W. It is immediate that the algebra morphism
kQ{ip) ¦¦ AV —> AW satisfies AQ(ip) ? di = dx ? AQ(ip).
Now let L and ? denote respectively the homotopy Lie algebras of (AV, d) and
(AW, d). Then Q(ip) dualizes to a Lie algebra morphism
? : L <— ?, {?,???) = (Q(tp)v;sx) ,
as follows from the compatibility of AQ(tp) and d\.
Finally, suppose X is a simply connected topological space with rational ho-
mology of finite type. In §21 (d) we defined the homotopy Lie algebra Lx =
7?»(??) ® h. On the other hand, X has a minimal Sullivan model
m: (AV,d) —> APL(X)
with its own homotopy Lie algebra L.
Now, by definition, sL = Hom(V, Jc). Identify sLx = nr(X) ® k by setting
sa = — (—l)dega571a, where 5, : ?*(?) ^» ?,-^??) is the connecting homo-
morphism for the path space fibration. In §13(c) we used the quasi-isomorphism,
m, to define a bilinear map ( ; ) : V ? ?*(?) —> Jk (Lemma 13.11). Define a
linear map ? : ?,(?) ® Jfe —>· Horn(V, A) by (??)? = (-l)de^a(v;a).
Theorem 21.6 The linear map
? : Lx > L
defined by ? (sa) = saa, a € Lx, is an isomorphism of graded Lie ?/
296 21 Graded (differential) Lie algebras and Hopf algebras
proof: Theorem 15.11 implies that ? is an isomorphism of graded vector spaces.
Hence so is ?. To check that ? preserves Lie brackets, recall that if ?,/3 € Lx
then their Lie bracket, as defined in §21 (d), satisfies
d^ [] d
On the other hand Proposition 13.16 states that
(v,[sa,s?\w) = (-l)deea+dee0+1 (d^sa^s?), ? € V .
Thus the Lie bracket in Lx satisfies
{dxv;sa,s?) = (-l)d**0+1(v;s[a,?\) ,
which is the defining condition for the Lie bracket in L. D
(f ) Differential graded Lie algebras and differential graded Hopf alge-
bras.
A differential graded Lie algebra (dgl for short) is a graded Lie algebra equipped
with a differential d satisfying d[x. y] = [dx, y] + (—l)deg x[x, dy]. Morphisms, sub-
algebras and ideals have the obvious meaning in the differential context. A chain
Lie algebra is a dgl in which L = {Lj}z>o- If Lo = 0, L is a connected chain Lie
algebra.
A (left) module for a dgl, (L, d) is a complex (V, d) together with a (left)
representation of L in V such that d(x ·?) = dx · ? + (—l)deg:rx · dv.
Let (L,d) be a dgl. Extend d uniquely to a derivation of square zero in the
tensor algebra TL and observe that this preserves the ideal I generated by the
elements ? ® y — (-l)de6x de&yy ® ? - [?,y]. Hence the universal enveloping
algebra UL = TL/I inherits a differential d, and with it the structure of a dif-
ferential graded algebra. The differential graded algebra (UL,d) is the universal
enveloping algebra of the dgl, (L,d) and is often denoted by U(L,d).
Note that (L, d)-modules are precisely the modules over the dga, U(L, d) and
so can be treated with the techniques of §6. Note also that if ? is a morphism
of dgl's then ? ? is a morphism of dga's.
As with dga's, the homology H(L) of a dgl inherits the structure of a graded
Lie algebra as follows: if ? and w are cycles in L representing homology classes
a and ?, then [?, ?] is the class represented by the cycle [2,11;]. If ? is a dgl
morphism and if (V, d) is an (L,d)-module then ? (?) is a morphism of graded
Lie algebras and [2] ·\?] = [? · ?] makes H(V) into an if (L)-module.
Finally, let (L, d) be a dgl and consider the inclusion ? : (L, d) —> U(L, d) as a
dgl morphism. Thus H(l) : H(L) —> H(UL) is a Lie morphism with respect to
the commutator bracket in the graded algebra H(UL). In particular, it extends
to a morphism of graded algebras, UH(L) —> H(UL).
Lie Models 297
Theorem 21.7
(i) The morphism UH(L) —> H(UL) is an isomorphism.
(ii) A dgl-morphism ? is a quasi-isomorphism if and only if ?? is a quasi-
isomorphism.
proof: (i) Recall the isomorphism 7 : AL —> UL of Proposition 21.2. Extend
d to a derivation in AL; then 7 is a morphism of complexes. Write L — ? ©
V @W where d = 0 in ? and d : V —> W. Then, as is noted in Lemma 12.5,
H(A(V © W)) = Jfe and so (??, 0) ^> (AL, d).
Identify ? = H(L) and observe that ?? A UH(L) —» H{UL) coincides
7
with the isomorphism ?? ? ? (AL) -^-> H(UL). Thus UH(L) A H{UL).
?(?)
(ii) The isomorphism of (i) identifies H(Utp) with UH(tp). But 7 iden-
tifies AH (?) with UH(ip) and so ? (?) is an isomorphism if and only if UH(ip)
is. D
Finally, we define differential graded Hopf algebras: these are simply graded
Hopf algebras together with a differential compatible with all the algebraic struc-
ture. It is immediate from the definition that U(L, d) is a differential graded Hopf
algebra. In §26 we shall see that so as Cm(UX;Jk) and establish an equivalence
(up to homotopy) between C*(fLY;l:) and a suitable U(L,d).
Exercises
1. Suppose ? G L and consider x2 G UL. Prove that x2 G L. Is it true that
xk € L for k > 3?
2. Let L be the quotient of the free Lie algebra L(a, 6) by the ideal generated
by the element [a, [a, b]] G L(a, 6). Prove that if ? and 6 are of even degree then
a2b + ab2 = 2a[a,b] in UL.
3. Let L = V 0 L" be the sum of two graded Lie algebras. Prove that UL =
UV ®UL".
4. Let F ~> ? ? ? be a fibration with 1-connected base and fibre. Prove
that if ?»(?) ® (Q> is onto then the graded algebra H*(UX,Q) is isomorphic to
H*(VY,Q) ® H.(CIF,Q).
5. Compute the homotopy Lie algebra Lx when A' = CPn, ? > 2, X = SpVSq,
X = CPp V CP? for p, q > 2, or X = CP°° V CP°°. Deduce if,(??,«© in each
case.
298 21 Graded (differential) Lie algebras and Hopf algebras
6. Let (A,d, e) be a differential graded augmented algebra. Deduce from §19-
exercise 1 that if A is commutative, then B(A) is a commutative differential
graded Hopf algebra with the shuffle product defined in §16-exercise 1 and the
usual coproduct. Determine the Hopf algebra B(Ik[x]/(x2)) when the degree of
x is even.
7. Let (A, e) be a graded augmented algebra. Prove that if A is commutative,
then TorA(Ik, Ik) is a graded Hopf algebra.
8. Prove that the adjoint representation (example 2) extends to a left represen-
tation of L in AL and that the morphism 7 of Proposition 2 is L-linear. Deduce
that UL is the direct sum of the L-modules j(AkL), k = 0.1....
9. Let 0 -> L' ~> L ~> L" ~> 0 be a short exact sequence of graded Lie algebras.
Define the Hopf kernel of Uf : UL -* UL" by HK(f) = {x G UL, (id ?
/)(?? - ? <g> 1) = 0)}. Prove that HK(f) is isomorphic to UL' and that UL =
UL' ®UL",
10. Let Ik be a commutative ring with unit and ? be the least prime, if any.
which is not a unit in Ik. Prove that (p — 1)! is a unit in Ik. Let (L,d) be a
differential graded Lie algebra such that Li = 0 for i < r. Prove that the natural
inclusion (L,d) -> (UL,d) induces injective maps Hn(L,d) -> Hn(UL,d) for
? < rp.
11. Let A' be a simply connected finite type CW complex. Supposing that
dimii»@X;Q) < 00 and that H+(X;Q) ? 0. Prove that X has the rational
homotopy type of a finite product of Eilenberg-MacLane spaces of the form
Ar(Q,2n).
12. Let V be an ?-dimensional Q-vector space concentrated in degree 2. Using
Poincare-Birkhoff-Witt, compute the dimension of the brackets of length 2r in
hv, for r > 1.
22 The Quillen functors C* and C
In this section the ground ring is a field h of characteristic zero.
A coalgebra of the form C = h ® {Cr!}z>2 will be called one-connected. Such
coalgebras are co-augmented by k. The object of this section is to construct
Quillen's functors
one-connected r , , .
c . connected chain
cocommutative ; > ,. . ,
, . . *~^ Lie algebras
chain coalgebras °-
and to establish the natural quasi-isomorphisms
C(C,(L,'dL)) A (L,dL) and C. (C(C,d)) ?- (C,d).
In §24 we shall see how this correspondence exhibits chain Lie algebras as an
alternative description for rational homotopy theory.
The passage from (L, ul) to C,(L, ul) is essentially inverse to the construction
of the homotopy Lie algebra of a minimal Sullivan algebra given in §21 (d) and
we make this remark precise in §23.
This section is organized into the following topics:
(a) Graded coalgebras.
(b) The construction of C*(L) and of C*(L; M).
(c) The properties of C*(L; UL).
(d) The quasi-isomorphism C*(L) ^» BUL.
(e) The construction of C(C, d).
(f) Free Lie models.
(a) Graded coalgebras.
Recall (§3(d), §19) that a graded coalgebra C is equipped with a comulti-
plication ? : C —> C <g> C and an augmentation ? : C —> h and that a
co-augmentation is an inclusion k c—> C so that ?A) = 1 and ?A) = 1 ® 1. For
such a coalgebra we write C = kere, so that C = k® C._ _ _
As described in §19, the reduced comultiplication ? : C —> C ® C is defined
by Ac = ?? — c ® 1 — 1 ® c. Its kernel is the graded subspace of primitive
ejements. Now set ?^0^ = id^, ?^ = ? and define the nth reduced diagonal
?(") = ' (? <g> id <g> ¦ · ¦ <g> id) ? A(n-V :C—>-C®---®C(n + l factors). We say
C is primitively cogenerated if C = |JkerA(n). Notice that if C = k®C>0 then
?
C is automatically primitively cogenerated.
300 22 The Quillen functors Cm and C
Finally, recall that C is cocommutative if ?? = ? where ? : C ®C —> C ® C
sends a ® b ·-> (-l)dee Q dee 66 ® a.
The main example, for us, of such graded coalgebras is the coalgebra AV
whose comultiplication ? is the unique morphism of graded algebras such that
A(v) = ? ® 1 + 1 ® ?. ? G V". It is augmented by ? : \+V —> 0, 1 m· 1 and
co-augmented by fo = A°V. It is trivially cocommutative. It is also easy to see
that kerii*") = A-nV, and so AV is primitively cogenerated.
Among the primitively cogenerated, cocommutative coalgebras (AV, d) has an
important universal property. Let ? : A+V —> V be the surjection defined by
Lemma 22.1 Suppose C = Jk ® C is a primitively cogenerated cocommutative
graded coalgebra. Then any linear map of degree zero, f : U —> V lifts to a
unique morphism of graded coalgebras, ? : C —> AV such that ?? |^- = /.
proof: Define fW : C ® - · - ® C —> Ak V by
/(Cl) ? · · · ? f{ck).
Recall that A(o^ = id ? and define ? by ?A) = 1 and
ceC.
(Since C is primitively cogenerated, this is a finite sum.)
To verify that ? is a coalgebra morphism, write A^k~^c = 5Zcf <g> · · · <g>
a
c?. Co-commutativity implies that ?^'^ = JZ ^Oi) ® ' ' ' ® c"(fc)j ^or eacn
?
permutation, ?.
Co-associativity implies that ?(?) = (?(?) ® ?(?)) ? ? for all p,q such that
? + q = k — 1. Finally, for any vi G V,
? · · · ? ?*) = (vi ® 1 + 1 ® ui ) ? · · · ? (vk ® 1 + 1 <8> ?
k
= E^ITF^II ? ±Mi)a'"aMp)®)M)
p=0 ^ V ^' CTgSfc
Combining these facts in a straightforward calculation gives (? ® ?) A = ??.
To prove uniqueness observe that any morphism maps kerA^1^ into V. Hence
if ? is a second morphism then for c € kerA^1^, ?? = ??? = f(c) = ??? = ??.
Suppose ? and ? agree in kerA^") and let c G kerA^n+1^. By co-associativity,
Ac G kerA(n) ® kerA(n). Thus Aipc = (? g> ?)?? = (? ® ?)Ac = ???. This
implies that (? — ?)? = (?? — ??)? = 0. ?
An analogue of Lemma 22.1 also holds for coderivations. Indeed, suppose
g : AkV —> V is a linear map of some arbitrary degree, and k > 1. Define
Lie Models 301
?9 : AV —> AV by
9g(vi A •••Avn) = ^2 ±9^1, A ¦¦¦ Avilc) Avi A-¦¦uil---vik---Avn.
(Here means deleted and ± is the sign given by v\ A ¦ ¦ ¦ A vn = ±vi1 A · · · ? Vik A
v\ A ¦ ¦ ¦ ujj · · · Vik ¦ ¦ ¦ A vn.) Note that ?9 decreases wordlength by k — 1.
Lemma 22.2 ?9 is a coderivation in AV. It is the unique coderivation that
extends g and decreases wordlength by k — 1.
proof: The coderivation property is a simple calculation. Uniqueness is proved
in the same way as in Lemma 22.1. D
(b) The construction of C,(L) and of C,(L;M).
Let (L,cIl) be a differential graded Lie algebra. The differential and the Lie
bracket determine coderivations in AsL given explicitly by (Lemma 22.2)
k
do(sx\ A ¦ ·· ? sxk) = — ^(—l)nisxi ? · · · ? sd^x,- ? - · · ? sx/.,
and
di (sxiA- ¦ -Asxk) = ]? (-l)degIi+1(-l)"ijs[xi, Xj]As.Ti · · ¦ satt-·· sxj · · -Asxk.
(Heren,· = ? degsxj, and sxiA- ¦ -Asxk = (—l)nijsxiAs
sxk. The symbol "means 'deleted'.)
Since d0 and d\ are coderivations of odd degree, g?o, dQd\ + dido and d\ are
also coderivations, decreasing wordlength by 0, 1 and 2 respectively. Moreover
dl(sx) = sd\x = 0;
(dodi + dido)(sx A sy) = (-l)de^s {dL[x,y] - [dLx,y] - {-l)d^*[x,dLy}) = 0;
d\{sx AsyA sz) = (-l)d^y+^s ([x> [y, z]] _ [[x,y],z] - (-l)degx degy^ [XjZjj)
= 0 .
Thus by the uniqueness assertion in Lemma 22.2, all three coderivations are zero.
Hi other words, (AsL, d = do + dy) is a differential graded coalgebra.
Definition The Cartan-Eilenberg-Chevalley construction on a dgl, (L, g^) is
the differential graded coalgebra
C.(L,dL) =
We often abuse notation and write simply C(L) for C*(L,di).
302 22 The Quillen functors C, and C
This construction is functorial: if ? : (E,d?) —> (L,cIl) is a dgl morphism
then C9 (?) is the dgc morphism ?? : As ? —> AsL, with Tp(sx) = ???.
Remarks 1 The construction C,(L) is a cocommutative co-augmented and
primitively cogenerated coalgebra.
2 For the construction for Lie algebras see Chevalley-Eilenberg [38]
and Cartan-Eilenberg [35]: for the differential graded case see Quillen ([135],
Appendix B).
3 The reader may have noticed two apparent coincidences: the first
between the construction above and the homotopy Lie algebra of a Sullivan
model (§21(d)); the second between the construction above and those in §19.
These are not coincidences. The first will be explained in §23 and the second in
§22(d), below. D
Next, we extend the construction above to the case with coefficients in a graded
(L,c??)-module, (M,d). Denote the differential in C(L) by d= d0 +d~i. Define
a complex (C*(L; M),d = do + d\) as follows:
• C*(L; M) — AsL <g> M as a graded vector space.
• d0 = d0 ® id + id ®d.
• di = d\ <8> id +?, where
k
9(sx\ ? · · · ? sxk <8> m) = /,(—l)niS?i ? · · · sx?· · · · ? sx/. ® ?,· ·??
k k
and m = ? (dee*i + 1) + ? (degxi + lXdegx,· + 1).
(Note that, as always, the tensor product of linear maps is defined by (/ <8>
5)(a® b) = (-l)d**'d**af(a)®g(b).)
As in the case of C*(L) we have dl = dod\ + dido — d\ = 0. Indeed ?% = 0
because d2 = 0, dido + dodi = 0 because d(x-m) = dLX-m + (—l)degxx-dm
and d\ = Q because [x,y]-m = x-y-m- (-l)degz degi'y ·? ·??.
(c) The properties of C,(L; [/?,).
Recall that (UL,d) = U(L,dL) is the universal enveloping algebra of the
dgl (L,di) — cf. §21(e). Multiplication from the left makes UL into a left
t/L-module, and hence into an L-module. Form the complex C*(L;UL) =
(AsL <g> UL,d), as described in (b).
Proposition 22.3 The inclusion k —> (AsL®UL,d) is a quasi-isomorphism.
Proposition 22.4 Right multiplication makes (AsL®UL, d) into a right semifre
(UL,d)-module.
Lie Models 303
proof of 22.3: In the proof of Proposition 21.2 we introduced the subspaces
UL(n) C UL spanned linearly by the monomials ?? ¦ --xk, x; € L, k < n. We
showed there that a linear isomorphism 7 : AL —> UL is given by x\ ? · · · Axk ?-4
^r ? ????(?) ' ' · xa(k) > and that it induces isomorphisms AkL -^ UL(k)/UL(k —
?
1)-
Now write AsL ® UL as the increasing union of the graded subspaces Ff. =
? A-lsL <g> UL{j). As usual, identify AsL <g> AL = A(sL © L). Then
^*(sL ? L) A Ffc, Jfe > 0,
and so induces isomorphisms Ah(sL ®L)
Next observe that the differential, d, preserves the spaces F/.. Moreover, be-
cause s[xi,Xj] has lower wordlength than sxi A sxj the corresponding terms in
the formula for d (see (b), above) disappear in Fk/Fk-i. Also, ?-7B/1 ? ··· ?
y le) — j(x ? yi ? · · · ? i/fc) € UL(k). These comments imply that id ®j is an
isomorphism of complexes
(Ak(sL®L),S) A (Fk/Fk^,d)
where ? is the derivation in the algebra A(sL ? L) specified by
6x = dx and Ssx = (-l)degx+1x - sdx, ? e L.
Trivially, H(sL ® L,S) = 0. Choose a basis of sL ® L of the form {???, iua}.
Then A(sL ? L, d) = (g) A(uQ, i«Q) and so ? (A(sL ? L), i) = Jk; i.e.
?
ii (Ak(sL ? L),i) = 0, k > 1. Because of the isomorphism above, ii (Fk/Fk-i,d)
= 0, k > 1. It follows by induction on k that ii (Fk/F0,d) = 0, k > I, and so
if ((AsL ® C/L)/Jk, d) = 0, as desired. D
proof of 22.4: It is immediate from the definitions that multiplication from
the right makes C*{L; UL) = AsL ® UL into a right (UL, d)-module. Moreover
„the subspaces M{k) = A-ksL 0 UL define an increasing family of submodules,
and
(M(k)/M(k - l),d) = (AksL,d0) ® (UL,d).
The two step filtration (kerd0) ® (UL,d) C (AksL,d0) ® (UL,d) exhibits
(M(k)/M(k - 1), d) as (UL, d)-semifree. Now Lemma 6.3 asserts that C,(L; UL)
is (UL, d)-semifree. ?
Notice that the construction Ct(L; UL) is functorial: if ? : (?, d) —i (L, d) is
a morphism of dgl's then
?*(?) ® ?? : (AsE ® UE, d) —> (AsL ® UL, d)
304 22 The Quillen functors C, and C
is a morphism of (UE, d)-modules, where UE acts on UL from the right via ? ?.
Moreover, because of the acyclicity (Proposition 22.3), C*(ip) ®Uip is automati-
cally a quasi-isomorphism.
Recall from Theorem 21.7 that ? is a quasi-isomorphism if and only if ? ? is.
Using the construction above we prove
Proposition 22.5
(i) If ? is a quasi-isomorphism then so is C*(<p),
(it) If ? = {?{};>() and L = {L,}i>o then ? is a quasi-isomorphism if and
only if C, (?) is a quasi-isomorphism.
proof: (i) Here Uip is a quasi-isomorphism (Theorem 21.7) and C,(ip) ®Utp is
a quasi-isomorphism because of Proposition 22.3. Since the modules Ct (E; UE)
and C,(L; UL) are semifree, Theorem 6.10 (ii) asserts that
(C«(y>) <8> Uip) ®?? k ¦¦ (As? <g> UE) <%UE Jk —> (AsL ® UL)
is a quasi-isomorphism. But this is precisely C,(?).
(ii) Conversely, suppose C,((/j) is a quasi-isomorphism. Consider
(UL, d) as a left (UE, d)-module via multiplication on the left by (Uip)a, a 6 UE.
Then
C.(tp)®id:C.(E;UL) ^Ct(L;UL)
is a morphism of right semifree (UL, d)-modules. Moreover (??(?) <g> id) ®ul Ik
is the quasi-isomorphism C* ((/>). Now Theorem 6.12 asserts (because ? and L are
concentrated in strictly positive degrees) that ?»(?) ? id is a quasi-isomorphism.
Hence H(C*(E;UL)) = Jk.
Now choose a (UE.d)-semifree resolution of left (U?, d)-modu\es, a : (M,d)
^ (UL,d), with M = {Mi}i>0. Since Ct(E;UE) is (UE,d)-semifree,
C*(E; UE)®ue — preserves quasi-isomorphisms. Furthermore, since M is semi-
free, — <8>ue A/ preserves quasi-isomorphisms. Thus
k ®?? ? 4^- C*(E; UE) ®?? ? ^^ C*(E; UE) ®UE UL = C.(E; UL);
i.e. H(k ®ue M) = k.
Define ? : (UE,d) —> (M,d) by ?? = a-m, where m is a cycle in Mo such
that H(a)[m] = 1. The calculation H (He ®?? ?) = k shows that k <%>?? ?
is a quasi-isomorphism, and hence so is ?, again by Theorem 6.12. Moreover,
H(a) is an isomorphism by construction. Thus H(U<p) = H(a) ? ?(?) is an
isomorphism. Now Theorem 21.7 asserts that ? is a quasi-isomorphism. D
We now return to the construction C,(L\UL). Recall from (a) above that
AsL is a cocommutative graded coalgebra and from §21 (e) that (UL,d) is a
differential graded Hopf algebra.
Lie Models 305
Proposition 22.6
(i) The tensor product of the diagonals in AsL and in UL makes C,(L;UL)
into a differential graded coalgebra.
(ii) The linear isomorphism 7 : AL -=» UL of Proposition 21.2 is an isomor-
phism of coalgebras. Hence C» (L; UL) = AsL <g> AL as a graded coalgebras.
(in) The module action (AsL®UL,d)®(UL, d) —> (AsL®ULxd) is a morphism
of graded coalgebras.
(iv) The quotient map — <S>ul k '¦ (AsL <g> UL, d) —> (AsL, d) is a morphism of
graded coalgebras.
proof: The tensor product of the diagonals in AsL and in UL certainly makes
AsL <8> UL into a graded coalgebra. Moreover the isomorphism 7 : AL -=> UL
of Proposition 21.2 is an isomorphism of graded coalgebras, as follows from the
relation
Aul(x\ •••in) = (x'i <8> 1 + 1 ®xi)---(xfc ® 1 + l<g>zjt) , x* € L .
To show that the differential is a coderivation denote the differentials in AsL =
C« (L) by do and d\ ; they are both coderivations. Since d is a coderivation in UL
(§21(e)) it follows that do = do® id + id®d is a coderivation. Moreover, recall
that d\ = d\ <g> 1 + ? where d\ is a coderivation and 9(sx\ ? · · · ? sxk <?> a) =
? ±sxi ¦ ¦ ¦ sii ¦ ¦ ¦ sxk <S> Xid. Since Am is an algebra morphism. Am(x{a) =
(xi ® 1 + 1 ® Xj)A(/?O. This implies (after a short calculation) that ? is a
coderivation.
The remaining assertions are self-evident. D
"(d) The quasi-isomorphism C,(L) -^ BUL.
As we observed earlier, the constructions Ct(L) and Ct(L:M) bear a strong
similarity to the bar constructions of §19. We can now make this precise.
Let (L,d) be a dgl and denote by BUL the bar construction on the dga
(UL.^d). As in §19, B(UL:UL) denotes the bar construction with coefficients in
UL, where UL acts on itself via left multiplication.
Now BUL is the tensor coalgebra TsUL on the suspension of the augmentation
ideal HZ c UL. The inclusion L C UL (§21 (a)) has its image in UL; hence we
may regard sL as a subspace of sUL.
Proposition 22.7 A natural dgc quasi-isomorphism
A : C.(L) ^ BUL
is given by A : sxi ?- · -Asx^. ?-» ^ ?^[sxCT(i)| · · · \sxa(ic)], where sxi ?- · -Asx/t =
S
306 22 The Quillen functors C, and ?
proof: A straightforward, if tedious, calculation verifies that A is a morphism
of dgc's. Similarly.
A ® id : (AsL ® UL, d) —> (BUL ® ?/L, d)
is a morphism of (UL, d)-modules. According to Proposition 19.2, H(BUL <g>
t/L) = 1; and (BUL®UL, d) is (?/L, d)-semifree. According to Propositions 22.3
and 22.4, (AsL <g> UL, d) has the same two properties.
Because H (BUL <g> ?/L) = Jk = H (AsL <g> ?/L), A <g> zc? is necessarily a quasi-
isomorphism. Because both are (UL, d)-semifree, Proposition 6.7 (ii) asserts
that (A <g> id) ®ul & is a quasi-isomorphism. But, trivially, (A <g> id) ®ul Jk = X.
D.
Remark Proposition 22.7 replaces the huge complex T(s~1UL) by the rel-
atively small complex AsL, which is a major computational advance. In the
classical case of ungraded Lie algebras without differentials, the smaller complex
in fact came first, as the dual of the left invariant forms on a Lie group. The bar
construction and the equivalence of Proposition 22.7 appear later in [118] and
[35].
(e) The construction L(C,d).
Recall that the free graded Lie algebra Ly is the sub Lie algebra of TV gener-
ated by V" (§21(c)) and that TV is the universal enveloping algebra of Ly. Thus
a dgl of the form (Ly, d) will be called a free dgl Note that this is a serious
abuse of language: (Ly ,d) is almost never a free object in the category of dgl's.
Recall first some basic facts about free Lie algebras Ly (cf. §21(c)). First,
Ly is the direct sum of the subspaces Ly of bracket length i, and Lv = V.
Second, any linear map / : V —> L (L a graded Lie algebra) extends uniquely
to an algebra morphism TV —> UL, which then restricts to a Lie morphism
Ly —> L, the unique extension of /. Finally any linear map g : V —> Ly
extends uniquely to a derivation ? of TV which then restricts to the derivation
of Ly uniquely extending g.
Let (Ly, d) be a free dgl. The linear part of the differential d is the differential
dy '¦ V —> V defined by dv — dyv 6 0 Ly . It suspends to a differential d in
sV : dsv = —sdyv.
Now consider the composite
? : C*(Ly ) = AsLy —> shy ?!" —> sV ? ]k,
where we have first annihilated A-2sLy and then s(Ljr ).
Proposition 22.8 The linear map ? is a natural quasi-isomorphism of com-
plexes,
(C.(Lv),d) A (sV ®k,d).
Lie Models 307
proof: It is immediate from the definitions that ? commutes with the dif-
ferentials. Moreover, since Uhy = TV, there is an analogous morphism ?' :
(BUhv,d) —> (sV(BJk,d) constructed in §19 just before Proposition 19.1. More-
over, Proposition 19.1 asserts that ?' is a quasi-isomorphism.
It is immediate that the quasi-isomorphism of Proposition 22.7, A : C» (Lv ) -^>
BUhy, satisfies ?'? = ?. Thus ? is a quasi-isomorphism. D
A prime (but by no means the only) way of constructing free dgl's is through
Quillen's functor, C, which is the analogue here of the cobar construction. The
functor C is constructed as follows. Let (C, d) = (U, d) ®Jk be any co-augmented
dgc, which is cocommutative. Form the cobar construction, Q.C — Ts~xC, as
described in §19. The differential has the form d = do + d\ with do preserving
s~1C and di : s~1C —> s~lC ® s~xC. Moreover, because C is cocommutative,
we can express d\ (s~1c) as a sum of commutators in the tensor algebra Ts~lC.
Indeed, write Ac = ?>j ® bt. Then also Ac = ?(-i)deeQi deg&,o. ^ o. jjence
(_l)(dega,-l)(degfc-l)s-l&i
This shows that di : s~xC —> ^s-iU c ?C~??). Because d\ is, in particular, a
Lie derivation it preserves Ls_i^. Hence so does d; i.e., (Ls_ic, d) is a dgl with
universal enveloping algebra flC
This construction is obviously functorial.
Definition The dgl (hs-i-g,d) will be called the Quillen consti-uction on the
co-augmented cocommutative dgc, (C, d) and will be denoted by C(C,d).
Theorem 22.9 Suppose (L = {Li}i>i,d) is a connected chain Lie algebra
and (C = k ? C>2,d) is a cocommutative dgc. Then there are natural quasi-
isomorphisms
? : (C, d) ^ C.C(C, d) and ? : CC.(L, d) A (L,d)
of dgc's (respectively, of dgl's).
proof: (i) Existence of ?. Write C = C>->. Thus ?(C,d) = i-s-ic anc*
= AsLs_i^. Thus by Proposition 22.8 we have a quasi-isomorphism of com-
plexes,
308 22 The Quillen functors C, and C
Now ss~lC = C, and a quick inspection shows that d is the original differential
in C:
However, g is not a coalgebra morphism. To obtain one, apply Lemma 22.1
to the inclusion
/ : C = ss-^ C sL^c C
noting that C is trivially primitively cogenerated because C = {Ci}i>2· This
gives a unique morphism ? : C —> AsLs_i ^ of coalgebras such that (? — f){C) C
A-2. We show first that ? is a dgc morphism, and then that it is a quasi-
isomorphism.
For simplicity, write L = Ls_! ^ and denote the differentials in L by d = do + ??
with dos~1c = —s~ldc and d\ reflecting the diagonal. Then write ? = ??? where
?? : C —> iVsL is the component of wordlength i. Let ? : A+sL —> sL be the
projection with kernel A-2sL.
Now observe that ?(?? — dip) — 0 or, equivalently, that ??? = ???? + dnp2-
Indeed, ???\? = do(ss~1c) = —sds~1c. In defining ?(C,d) we gave an explicit
formula for dsc. Thus if, Ac = ? c; ® c'v then
On the other hand, ?\?? = ss^ and (cf. Lemma 22.1)
dupoc = ?? I ^J2ss~1ci A ss~ic'i j
It follows that ??? =
Put ? = ?? — ??. We have just seen that fo = 0. The coderivation property
implies that (? ® ? + ? <S> 0)? = ??, where ? denotes both reduced diagonals.
By coassociativity, ? : kerA^"^ —> kerA(n~^ ®kerA("\ where ?(?) is the
nth reduced diagonal as defined in §22(a). Assume by induction that ? vanishes
on kerAt"-1). Then for c € kerA(n\A0c = 0 and 9c = ??? = 0. It follows by
induction that 0 = 0; i.e. ?? = ??.
Finally, note that ?? = id. Since ? is a quasi-isomorphism, so is ?.
_ (it) Existence of ?. Put C = C+{L) so that C = A+sL. Define
? : s~1C —> L by setting a(s~lsx) = ? and aCs'^a) = 0, ? 6 A-2sL. Then since
Ls_ic is a free Lie algebra., ? extends to a unique Lie morphism -? : i-s-ic —> L.
As in (i) we have to check that ? commutes with the differentials and that ?(?)
is an isomorphism.
The linear map ? = ?? - ?? satisfies 0[a,b] = [??,??] + (~l)desa[ipa,0b],
a, b 6 Ls_!^. Since this Lie algebra is generated by s~*C it is enough to check
that ? vanishes there. This is a straightforward computation from the definition
of the differentials, as in (i).
Lie Models 309
Let ? : Ct(L) ^> C»Ls_!c be the dgc quasi-isomorphism constructed in part
(i) of the theorem (for general cocommutative dgc's). A quick computation shows
that 0*{?)? = id. Hence Cm(ip) is a quasi-isomorphism, and then so is ? by
Proposition 22.5. ?
Corollary Suppose a : (C,d) —> (C',d) is a morphism between dgc's satisfying
the hypotheses of the theorem. Then a is a quasi-isomorphism if and only if C(a)
proof: The theorem identifies H(a) with ? (CtC(a)). Now apply Proposi-
tion 22.5. D
(f) Free Lie models.
In §22(e) we introduced the free dgl's (L\.-,d). Thus (Ly,d) is a connected
chain Lie algebra precisely when V — {Vi}z>1. These play the same role within
the category of connected chain Lie algebras that Sullivan algebras play in the
category of commutative cochain algebras, and for the same reason: the under-
lying algebraic object is free.
In particular we make the
Definition A free model of a connected chain Lie algebra (L, d) is a dgl quasi-
isomorphism of the form
m : (Lv, d) ^ (L, d)
with V = {Vi}4>1.
There is a simpler approach, however, which extends any morphism 7 : (Lh' , d) —1
(L, d) of connected chain Lie algebras to a free model: (? is the obvious inclusion)
(Lw@v,d) ~ (L,d)
?\ ? B2·10)
(Uv,d)
Indeed, suppose for some r > 0 that Hi(j) is an isomorphism for i < r and
surjective for i = r. Extend 7 to
7' : (Lu'©v,.+1, d) —> (L,d)
by requiring that
310 22 The Quillen functors C, and ?
• The elements dv, «el^!,,, are cycles in hw representing all the classes in
• The elements in V"+i are cycles and are mapped by 7 to cycles representing
all the elements in coker #,.+1G).
Then ??(?') is an isomorphism for i < r and surjective for i = r + 1. Iterate this
procedure to construct B2.10). D
Free Lie models of the form B2.10) are analogues of the relative Sullivan
models of §14. This motivates the
Definition A free Lie extension is a morphism ? : (Liv, d) —> (Lwev, d) of
free connected chain Lie algebras, in which ? is the obvious inclusion.
Suppose now given a commutative diagram
(Lw,d) ^^ (E,d)
?
(Uv&v,d) > (L,d)
of morphisms of connected chain Lie algebras. Assume ? is a free extension and
? is a surjective quasi-isomorphism.
Proposition 22.11 With these hypotheses a extends to a morphism
? : (Lw®v,d) —> (E,d) such that ?? = ?.
proof: Identical with that of Lemma 12.4, except that the induction is in the
degree of the elements in W. ?
Suppose next that (Ly, d) is a free connected chain Lie algebra. Then d
decomposes uniquely as the sum of derivations d; : V —> L^+1 . The differential
d0 : V —> V is the linear part of d, as defined in (e) above.
Similarly, if ? : (Lw, d) —> (Ly, d) is a morphism of free connected chain Lie
algebras then the linear part of ? is the chain complex morphism
defined by ? - ?0 : W —* L^-2).
Proposition 22.12 If ? : (h\v,d) —> (Lv,d) is a morphism of free connected
chain Lie algebras then
? is a quasi-isomorphism <=$¦ ?? is a quasi-isomorphism.
Lie Models 311
proof: According to Proposition 22.5 and Proposition 22.8 respectively,
? is a quasi-isomorphism <=> C, (?) is a quasi-isomorphism
<?=>¦ ?? is a quasi-isomorphism.
Definition A free connected chain Lie algebra (Ly, d) is minimal if the linear
part do of the differential is zero. In this case a quasi-isomorphism
m : (Lv,d) -=* (L,d)
is called a minimal free Lie model.
Theorem 22.13 Every connected chain algebra (L,d) admits a minimal free
Lie model
m:(Lv,d) A (L,d)
and (Ly, d) is unique up to isomorphism.
proof:" Theorem 22.9 provides a surjective free model, which we write as
Decompose W = V ®U ® doll with d0 = 0 in V and do : U ^> doll.
Next, let / c Liy be the Lie ideal generated by U and by dU. Thus / is
preserved by d and (Liy //, d) is a quotient connected chain Lie algebra. Denote
the quotient map by ? : (Lw, d) —> (h\v //, d).
It is an elementary exercise in algebra to verify that the inclusions of V,
dU and U into Liy extend to an isomorphism ? (V ®dU®U) -=^> TW (filter
by the ideals T-k). Hence Lw = Ly © / and ? restricts to an isomorphism
Ly —> Liv//. Use this to identify (Ljy //,d) as a free chain Lie algebra (Ly, J).
It is straightforward to check that the linear part of ? is the linear map
?? : W —> V which is the identity on V and zero in U and doU. Since
dO?Oi; = ?>odou = 0 it follows that (Ly,d) is minimal. Moreover ?(?0) is an
isomorphism and hence ? is a quasi-isomorphism (Proposition 22.12). Finally,
Proposition 22.11 allows us to lift id^v through ? to obtain ? : (Ly,d) —>
(h\v, d) such that ?? = id. Then
?? = ?? : (Ly,d) ^> (L,d)
is a minimal Lie model.
For uniqueness, let m' : (Ly,<f) -^> (L,d) be any other minimal Lie model.
Lift m' through the surjective quasi-isomorphism ?, and compose with ?. This
gives a quasi-isomorphism
312 22 The Quillen functors C, and ?
between minimal free connected chain Lie algebras.
Now Proposition 22.12 asserts that ?(??) is an isomorphism. But d0 and
d'o are zero, so ?? itself is an isomorphism. Now apply Proposition 22.12 to
? : (Lv,0) —> (Lv,O) to conclude that ? is an isomorphism. D
Notice that the last paragraph of the proof of Theorem 22.13 in fact establishes
Proposition 22.14 A quasi-isomorphism between minimal free connected chain
Lie algebras is an isomorphism. D
Exercises
1. Prove that if deg ? = 2n - 1, ? > 1, then ? : ? (?) -4 ?? <g> T(u) defined by
ip(v2k+1) = ? <8> uk and <p{v2k) = 1 ® uk is an isomorphism of coalgebras (see
§3-exercise 4). Deduce that
Ht(US2n;Q) = H*(Sn;Q) ® Ht(US4n~1;q).
2. Let ?,~? : (Lv ,d) -4 (L,d) be two morphisms of differential graded algebras.
A degree /c-linear map ? : Lv -4 L is an (?, ?)-derivation if it satisties ?([?, y]) =
[??,???] + (-l)d'g ?[??, Oy]. We write ? ~ ? if there exists an (?, ^-derivation
of degree 1 satisfying [?, d] = ? - ?. Prove that ~ is an equivalence relation.
23 The commutative cochain algebra, C*(L,dL)
In this section the ground ring is a field k of characteristic zero. In particular,
we write #„(-) and H*{-) for Hm(-;Jk) and #·(-; Jfc).
In §22 we constructed the dgc C*{L,cIl) associated with a dgl, (L,di). Here
we dualize that construction to obtain a commutative differential graded algebra,
C'(L,dL).
When L is a chain Lie algebra, C*(L,di,) is a cochain algebra, and so the full
Sullivan machinery of Part II may be applied. In particular we can use chain
Lie algebras (L,di,) to model topological spaces A" by requiring that C*(L,dL)
be a model for Apl{X). This is carried out in §24.
This section is organized into the topics
(a) The constructions C*(L, cIl) and ?{A,d)·
(b) The homotopy Lie algebra and the Milnor-Moore spectral sequence.
(c) Cohomology with coefficients.
(a) The constructions C*{L,di), and C^^y
Again, let (L,di,) be a dgl. Dual to the construction C»(L, c2l) we define the
differential graded algebra, C*(L,dL), by
C*(L,dL) =Eom(C,(L,dL),Jk)
as described in §3(d). Thus multiplication and the differential are given by
and Df)() (l)d*'/(fc) ''* ???
(When the differential in L is clear from the context we may abuse notation and
simply write C*(L) and C»(L), as in §22.)
This is entirely analogous to the construction in §5 of the singular cochain
algebra on a space X as the dual of the singular chain coalgebra. However here
we have the crucial fact that C*(L) is a commutative dga, because C»(L) is
cocommutative.
Suppose now that (L,dL) is a connected chain Lie algebra. Then C»(L) =
AsL = k © {Ci}i>o. It follows that C*(L) is a cochain algebra and so the
machinery of Sullivan models (Part II) can be applied. We shall see now that
if L is a connected chain Lie algebra and if each Li is finite dimensional then
C'(L) is, itself, a Sullivan algebra (§12).
Indeed, the decomposition AsL = ®AksL defines a surjection AsL —> sL
k
(not compatible with the differential in AsL), which dualizes to an inclusion
(sL)& = Hom(sL, Jfc) <—> C*(L) .
314 23 The commutative cochain algebra, C*(L,cIl)
Since C*(L) is commutative this extends uniquely to a morphism of graded
algebras
Lemma 23.1 // (L,d) is a connected chain Lie algebra and each Li is finite
dimensional then
? : A(sLY A C*{L)
is an isomorphism of graded algebras, which exhibits C*(L) as a Sullivan algebra.
proof: Let y, = sx{ be a basis for sL and let vj be the dual basis for (sL^ :
(vj,yi) = o{j. live (sL)> and ? € Ap(sL)* then
(???,?/i, A---Ayip+1) = (?®?,?^?? ? · · · Ayip+l)) , and
C= E(-l)de6^ de6*(v,yi.)(<e,vilA-~Vi.-~Ayip+l).
It follows that
(?*1 ? ¦ ¦ · ? ?*- ,j/?» ? · ¦ · ? yf1) = ??! ¦ · ¦ knl
(where ki < 1 if |vi| is odd) and that vf1 ?· · ·???" evaluates all other monomials
to zero. Since char h = 0 this implies that ? is an isomorphism, because C»(L)
is finite dimensional in each degree.
Now ? identifies C*(L) as a cochain algebra of the form (AV, d) with V =
{]/p}p>2. Such a cochain algebra is always a Sullivan algebra. Indeed define
V@) CVA) C ··· by V@) = VDkevd and V[k) = VDd'1 (AV(k-1)). Since
(AVK = F3, d(F2) C F3 and V2 C F(l) dj^ffc). Suppose V^" cU^(fe).
?· ?-
For ? € F", civ = w + ? with w 6 Fn+1 and ? 6 AV-n~l. Thus B? = -?? 6
? ( (J V{k) ) and so ? € (J F(A;). But then also ? ? (J F(A;) and it follows that
V = \JV(k). a
k
If F and W are graded vector spaces of finite type then an isomorphism
W = Vi defines a pairing ( ; ) : W ? V —> L· which in turn determines an
isomorphism V = WK In the case we say V and W are dual graded vector
spaces with pairing ( ; ).
Proposition 23.2 Suppose (L.dL) is a connected chain Lie algebra of finite
type and each Li is finite dimensional. Then
(i) C*(L, d[^) — (AV,d) and V and sL are dual graded vector spaces.
(ii) d = do+di is the sum of its linear and quadratic parts (§12(a) and §13(e)),
and
Lie Models 315
(dov;sx) = (-l)de^v(v;sdLx) and (dlV;sxAsy) = (-l)de*v+l(v;s[x,y]) .
Conversely, suppose (AV, d) is an arbitrary Sullivan algebra such thatd = do +
d\ and V = {Vp}p>2 with each Vp finite dimensional. Then a connected chain
Lie algebra {L,di) is determined uniquely by the condition (AV,d) = C*(L,di).
proof: The first assertion follows from Lemma 23.1 and the definition of the
differential in C»(L,c2l). For the second assertion let L be the desuspension of
Hom(F, k) and use the formulae above to define d^ : L —> L and [, ] : L ? L —>
L. The equation d2 = 0 reduces to dj = 0, dodi + dido = 0 and ds = 0. These
translate respectively to: [ , ] is a Lie bracket (§21(e)), d^ is a Lie derivation
and di = 0. D
Finally suppose that (.4, d) is a commutative cochain algebra in which ,4 =
Jk(B A-2 and each A1 is finite dimensional. Let (C,dc) = Hom(J4, Jfc), recalling
that dc is the negative dual of d (§3(a)). Because .4 has finite type, the multi-
plication in A dualizes to a comultiplication in C, which makes (C,dc) into a
cocommutative differential graded coalgebra with dual cochain algebra (A,d).
Apply the functor C of §22 to this dgc and, abusing notation, denote the result
by ?{A,dy-
?(A,d) = ?(C,dc) ¦
Note that C(A,d) ls a connected chain Lie algebra, finite dimensional in each
degree. Thus the quasi-isomorphism ? of Theorem 22.9 dualizes to a qu'asi-
isomorphism of commutative cochain algebras
thereby exhibiting C* (C^d)) BS a functorial Sullivan model of (A,d).
Example 1 Graded Lie algebras.
Suppose L = {Li}i>i is a graded Lie algebra and each Li is finite dimensional.
Then Proposition 23.2 reduces to
in which sL and V are dual graded vector spaces and d\ is purely quadratic.
Conversely, any commutative cochain algebra of the form (?V, di ) with V =
{ Vp}p>2> each Vp finite dimensional and d\ purely quadratic determines a graded
Lie algebraL by the requirement that (AV,d\) = C*(L,0).
Next notice that Propositions 22.3 and 22.4 identify C*(L;UL) as an exact
sequence
0 <— k^UL^- sL®UL^- A'2 4
of right t/L-modules. In other words (cf. §20(a)) this is a free f/L-resolution
of the trivial f/L-module, Jk. On the other hand, it is immediate from the
definitions that C*(L) = HomuL (C*(L;UL),]k), and so
).. a
316 23 The commutative cochain algebra, C*(L,Al)
Example 2 Free graded Lie algebras.
Suppose ? = {?'i}!>2 is a graded vector space of finite type and (H, 0) is the
commutative cochain algebra with zero differential defined by
H = h@E and E-E = 0 .
The dual graded coalgebra has the form C — k@C with ? = 0 : C —>~C& C.
Thus the differential in ?(c,o) is zero (§22(e)). In other words, if W is the graded
vector space defined by W,· = Horn (?'+?,?) then
?(H,0) = ?(C,0) = CUv j 0) .
Thus in this case dualizing Theorem 22.9 provides a cochain algebra quasi-
isomorphism
and C*.(Lw, 0) is a minimal Sullivan algebra with purely quadratic differential.
In other words, this is the minimal Sullivan model of (H,0), and so we recover
the construction of Example 7, §12(d). ?
Example 3 Minimal Lie models of minimal Sullivan algebras.
Suppose (AW,d) is a minimal Sullivan algebra and that W = {W!}->, is
a graded vector space of finite type. Let (Ly ,9) be a minimal Lie model of
?(AW,d) (Theorem 22.13). Then by Proposition 22.5 the quasi-isomorphism ? :
(Ly ,9) -=> ?(AW,d) induces a quasi-isomorphism C*(a) which then dualizes to a
quasi-isomorphism C*(a) : C* (Ly ,9) <=- C* (?(\w,d))· On the other hand, as
remarked above, we have a quasi-isomorphism C* (?(Aw,d)) -^ (AW,d).
This has a homotopy inverse (AW,d) -^ C* (?(\w,d)) (Proposition 12.9), and
the composite
?: (AW,d) A C*(Ly,9)
exhibits (AW,d) as a minimal model for C* (Ly,9): z/(Ly,9) is a minimal Lie
model for (AW,d) then (AW, d) is a minimal Sullivan model for C* (Ly ,9).
Recall further that C* (Ly,9) is itself a Sullivan algebra (AZ,d) with ? =
Horn (sLy, Jc). Thus dividing by A~2W and A-2Z yields a commutative diagram
H+(AW,d) —^-+ H+(C*(U>,d))
<
W =—- Horn (sH (Lv, 9), jfe) ,
where Q(tp) is the linear part of ? and is a quasi-isomorphism because ? is
(Proposition 14.13).
On the other hand, Ly = V ? [Ly, Ly ] and Im 9 C [Ly, Ly ]. Thus dividing by
[Ly,Ly] is a surjective chain map ? : (Ly,9) —> (^»0). The quasi-isomorphism
Lie Models 317
C>o (Lv,<9) -=» sV of Proposition 22.8 converts the inclusion shy —> C, (Ly)
into ??. Its dual therefore converts the dual of ?? into the surjection A+Z —> ?.
Thus it identifies Horn (sH(?), k) with the map ?' in the diagram above, and we
obtain the commutative diagram
H+(AW,d) ^— Hom(sV,Jfe)
Horn (sH (Ly ) , Jfe)
>2
Example 4 Sullivan algebras (AW,d) for which H2k(AW,d) =0, 1 < k < n.
Here we consider minimal Sullivan algebras (AW,d) such that W = {
is a graded vector space of finite type. The surjection (A+W,d) —> (W,0) with
kernel A^2W induces a linear map ? = {?? : HP(AW) —> Wp}. We shall use
Lie models to show that
H'2k(AW,d) = 0, 1 < k < ? => Cp is injective , ? < 2n + 1 .
In fact, let (Ly,<9) be a minimal Lie model for ?(Atv,d) (Theorem 22.13).
Then (Example 3), H+(AW,d) = Eom(sV,Je) as graded vector spaces. Thus
Vofc-i = 0, 1 < k < ? and L\/<2n is concentrated in even degrees. For degree
reasons the differential in Ly<2n is then zero.
Recall from Example 3 that ? : (Ly,<9) —> (KO) is the surjection with kernel
[Ly,Ly]. Since d vanishes in degrees < 2n, ^G7) is surjective for i < 2n.
It follows that Horn (sH(?),&) is injective in degrees ? < 2n + 1. Example 3
identifies this dual with ?; i.e. ?? is injective, ? < 2n + 1. D
(b) The homotopy Lie algebra and the Milnor-Moore spectral se-
quence.
Consider an arbitrary minimal Sullivan algebra (AW,D) such that W =
{Wp}p>2 and each Wp is finite dimensional. Then D = Di + Do ? with
Di : W —> Ai+1W. Associated with (AW, D) is its homotopy Lie algebra ? (as
defined in §21(e)), and it is immediate from that definition that
(AIF,?>i) =C*(E)
(where ? is regarded as a dgl with zero differential). Indeed, as observed in
Proposition 23.2, this relation characterizes E.
Next suppose (L,cil) is a connected chain Lie algebra with each L/ finite
dimensional. Let
m: (AW,D) ^ C'(L,dL)
be a minimal Sullivan model and let ? be the homotopy Lie algebra of (AIT7, D).
As in Proposition 23.2, write C*(L,Bi,) = (AV,d0 + di) with V dual to sL. Let
Q{m) : W —> V be the linear part of m (§12(b)). Then d0Q(m) = 0.
318 23 The commutative cochain algebra, C*{L.d,L)
Proposition 23.3 With the hypotheses above, Q(m) induces an isomorphism
W -^ H(V, do). Its desuspended dual is an isomorphism of graded Lie algebras,
H(L) A E .
proof: Use Theorem 14.9 and Theorem 14.11 to extend m to an isomorphism
of the form
(AW, D) ® (A(U © SU), ?) A (AV, d0 + dx)
with ? : U -^ OU. It follows that Q(m) induces an isomorphism
AH{Q{m)) : (AW,D{) A (AH(V,do),H(dx)) .
Now V is dual to sL and do is dual (up to sign and suspension) to c2l. This
identifies H(V,d0) as the dual of sH(L) and (AH(V,do),H(di)) as C (H(L),0).
Since (AW,Di) = C*(E,0), the 'desuspended' dual of H(Q(m)) is an isomor-
phism from H(L) to the homotopy Lie algebra E. D
Remark The effect of replacing C*(L) by (AW,D) is to get rid of the linear
part do and to convert d\ to Dx = H(d\). However we 'pay' for this simplification
through the addition of (possibly infinitely many) higher order terms Di, ?>3,...
in the sum D =
Finally, consider a general Sullivan algebra (AV,d) in which d = do + di
• · ·, with di : V —> Ai+lV. Filter (AV, d) by the decreasing sequence of ideals
FP = (AV)+ (AV)+ , p>l,
? factors
and set F° = AV. Then (independently of the choice of generating space V)
Fp = A-PV, and so this is called the wordlength filtration. It determines a first
quadrant spectral sequence (E{,di): the Milnor-Moore spectral sequence of the
Sullivan algebra. Any morphism ? : (AV, d) —> (AV',d') of Sullivan algebras is
automatically filtration preserving and so induces a morphism ??(?) of Milnor-
Moore spectral sequences. It is immediate from the definition that
(Eo,do) = (AV,d0) and ?0(?) = AQ{ip)
where Q(ip) : V —> V is the linear part of ? (§12(b)).
Next, let
m: (AW,D) ^ (AV,d)
be a minimal Sullivan model (Theorem 14.12) and suppose W = {Wp} and has
finite type. It follows from Theorem 14.9 and Theorem 14.11 that m extends to
an isomorphism
(AW, D) ® (A(U © OU), ?) -=> (AV, d)
Lie Models 319
with ? : U -^ 5U. Since this isomorphism and its inverse preserve wordlength
filtrations it induces an isomorphism of Milnor-Moore spectral sequences. In
particular, if ? is the homotopy Lie algebra for (AW, D) then
(E0,dQ) ? (AW,0)®(A(U®SU),S) , and
(E^dy) .? (AW,Dl) = C*(E) .
Thus the Milnor-Moore spectral sequence converges from
E2 = ExtUE(]k,]k) =*> H(AW,D) .
Example 1 C*(L,dL).
Let L be a connected chain Lie algebra with each Li finite dimensional. Then
the homotopy Lie algebra of C*(L, <2l) is just H(L) (Proposition 23.3) and so
the Milnor-Moore spectral sequence for C*(L, c2l) converges from
E2 = Extc/^i) (A, k) => HC* (L, dL) . ?
Example 2 Topological spaces X.
Suppose (AW,D) is the minimal Sullivan model for a simply connected topo-
logical space X with rational homology of finite type. Then ? (AW, D) =
H*(X;]k), H*(QX;]k) = ULx and ?? is isomorphic to the homotopy Lie alge-
bra of (AW,D). (This follows, respectively, from Corollary 10.10, Theorem 21.5
and Proposition 21.6.)
Thus the Milnor-Moore spectral sequence for a minimal Sullivam model of X
converges from
E2 = ExtH.{ii
(c) Cohomology with coefficients.
Suppose given a graded Lie algebra L and a right L-module M; i.e., M is a
right t/X-module. We say this defines a right representation of L in M. Denote
the action of ? € L on ? € M by ? · ?. Since (cf. §3(d) and Propositions 22.4
and 22.6) C*(L;UL) is a C,(L)-comodule and a right t/L-module and these
structures are compatible,
C*(L; M) = Homc/L (C*(L- UL), M)
is a C*(L)-module. Since C*(L\UL) is a f/L-projective resolution of h (Exam-
ple 1, §23(a)) we may identify
H (C*(L;M)) - Extudk M) . B3.4)
320 23 The commutative cochain algebra, C*(L,di)
The cohomology of this complex is called the Lie algebra cohomology of L with
coefficients in M'.
Now suppose that L = {Li}i>1 and that each Li is finite dimensional. Then
each Ck{L) is finite dimensional. Suppose further that M = {Ml}i>p some
? ? ? and use the convention Ml = M~i to write M = {??;}?<_?. Thus if
a : C»(L) —> M is a linear map, it will vanish on C>_|a|_p. It follows that
there are natural isomorphisms of graded vector spaces
C*(L;M) = RomUL (C.(L)®UL,M)
= Eom (C.(L)tM)
(93 ?)
= C*(L)®M .
Write C*{L) = (AV,d), where V is dual to sL and
(du; sz ? sy) = (-l)deg?/+1(v; s[x,y])
(Example 1, §23(a)). Then B3.5) identifies C*(L;M) as the (AF,d)-module
given by
C'(L;M) = (AV®M,d)
with the (AV, d)-modu\e structure simply multiplication on the left. Thus the
differential is determined by its restriction d : M —> V <8> M. If we identify
V <8> M = Hom(sL,M) via (v ® z: sx) = {-I)de&z^d^x+I'>{v,sx)z then this
restriction is given by
(dz;sx) = (-i)d*e*+deexz.x , z?M, xeL, B3.6)
as follows immediately from the definition of the differential in C,(L;—) at the
end of§22(b).
Conversely suppose given a (AV, d)-modu\e of the form (AV <8> N, d) in which
d-.N^r V® N. Then B3.6) defines a bilinear map ? ? L —> ? and it follows
easily from the equation d2 = 0 that this is a right representation of L in TV. By
construction, if ? = {??}?>? then
Exercises
1. Let M be a L-module and assume that L and M are graded vector spaces of
finite type. Prove that the graded dual M* is also an L-module and that there
exists a quasi-isomorphism (C*(L; M))* —> C*(L;M#).
2. Let L = L\ © L2 be the direct sum of two differential graded Lie algebras.
Prove that C"\l) = C*(LX) ® C*(L2).
Lie Models 321
3. Let (L(V),d) be a free differential graded Lie algebra. Denote by (L(V),d)
and (L(V"),d) two copies of (L(V),d). Prove that there is a quasi-isomorphism
? : (h(V © V" © sV),D) -+ (L(V),d) with ?{?') = ?(?") = ? for any ? 6 V,
D(sv) -?' + ?" € L^2(V ? V" © sV), and </>(sF) = 0.
4. Two morphisms of differential graded Lie algebras /,g : (L(V),d) ->· (L, <i)
are homotopic, / ~ p, if there is a morphism of differential graded Lie algebras
F : (L(V © V" © sV),D) -»· (L,d) satisfying F(u') = /(u) and F(v") = g{v).
Prove that the homotopy relation is an equivalence relation.
5. Prove that two morphisms of differential graded Lie algebras/, g : (L(V),d) ->·
(L,d) are homotopic in the sense of exercise 4 if and only if they are equivalent
in the sense of §22, exercise 2.
6. Assume / ~ g : (L(V),d) -+ (L,d). Prove that C(f) ~ C'E).
7. Consider the diagram of differential graded Lie algebras
(Lud,)
(h(V),d) A (L2,d2)
where V = {V^};>0 and dim Vi < co. Prove that if ? is a quasi-isomorphism
then there exists a morphism ? : (L(V),d) ->· (Li,di) such that ?? ~ ?.
8. Let h : (L(F),d) -»· (L(W')>cQ. and /,5 : (L(H7),^) -»· (^,«0 be morphisms
of differential graded Lie algebras. Assume that ? is a quasi-isomorphism and
that fh ~ gh. Prove that / ~ g.
9. Let /, g : (AV, d) —>¦ (A, d^) be morphisms of commutative differential graded
algebras. Suppose that / ~ g. Prove that ?(/)
10. Let L be a finite dimensional graded Lie algebra concentrated in even
degrees. Prove that there exists an integer ? such that dim HnC*(L, Ik) = 1
and dim HkC(L, Ik) = 0, k > n.
24 Lie models for topological spaces and CW
complexes
The ground ring in this section is a field Jc of characteristic zero.
The fundamental bridge from topological to algebra used in this book is Sul-
livan's functor
topological spaces — >· commutative cochain algebras ,
constructed in §10. Thus an algebraic object is a model for a topological space
X if it is associated with the cochain algebra Apl(X)·
For example (§12) a Sullivan model for X is, by definition, a Sullivan algebra
(AVx,d) together with a quasi-isomorphism
m:(AVx,d)-Z>APL(X) .
Analogously, we make the
Definition Let X be a simply connected topological space with rational ho-
mology of finite type. A Lie model for X is a connected chain Lie algebra (L, di,)
of finite type equipped with a dga quasi-isomorphism
m:C*(L,dL) A APL(X) .
If L = Lv is a free graded Lie algebra we say (L, <2?,) is a free Lie model for X.
If ? : C*(E,dE) -^> Apl{Y) is a Lie model for a second topological space Y
then a Lie representative for a continuous map / : X —> y is a dgl morphism
<p:(L,dL)—f (E,dB)
such that mC*(ip) ~ Api(f)n (as defined at the start of §12).
Example 1 The free Lie model of a sphere.
In the tensor algebra T{v) on a single generator, v, the free Lie subalgebra
h(v) is given by
lev if deg ? = 2n
hv @ h[v,v\ if deg?; = 2n + 1.
Thus the cochain algebra C*(L(w)) is given, respectively, by
(A(e),0) , dege = 2n + 1
(?(?,?'), de' = e2) ,dege = 2n + 2.
The reader will recognize these as the minimal Sullivan models for spheres
constructed in Example 1, §12(a). In other words, we have for all ? > 1 a
quasi- isomorphism
C*(L(u)) -?* APL(Sn+1) , deg ? = ? ,
Lie Models 323
which exhibits (L(i;),0) as a minimal free Lie model for Sn+1. D
As with Sullivan models we often abuse language and refer simply to {L,<1l)
as a Lie model for X. Lie models provide a description of rational homotopy
theory that is different from but equivalent to that provided by Sullivan models.
In particular, if we restrict to simply connected topological spaces with rational
homology of finite type then
• Every space X has a minimal free Lie model, unique up to isomorphism,
and every continuous map has a Lie representative.
• Every connected chain Lie algebra, (L,cIl) of finite type, and defined over
Q, is the Lie model of a simply connected CW complex, unique up to ra-
tional homotopy equivalence.
If (L, A1) is a Lie model for X then there is a natural isomorphism H(L) -^»
7?,(??) <8>z k of graded Lie algebras (§24(b)), which is in a certain sense 'dual'
to the isomorphism H(AVx) -^ H*{X;k) in the context of Sullivan algebras.
The first isomorphism suspends to an isomorphism
sH{L) ? ?.??®* ,
which (up to sign) converts the bracket in H(L) to the Whitehead product in
?» (?) ®k.
Finally, given any free rational chain Lie algebra (Ly, d) (connected of finite
type) we shall in (d) below, construct a CW complex X for which (L\.·, d) is a
Lie model. Here
• For each ? > 2, the ?-cells D? of X correspond to a basis {va} of Vn-i.
• The sub dgl (Ly<n ,d) is a Lie model for the ?-skeleton Xn.
• The homology classes [dva] e ? (hv<n_1) correspond to the homotopy
classes [fa] e ??_?(,??_?) <8> ifc of the attaching maps fa : S?~l —> Xn-\-
In this case (Ly, d) will be called a cellular Lie model for X.
Throughout this section we shall use the properties of Sullivan algebras (§12,
§14), often without explicit reference. For example, suppose (L,dL) is a Lie
model for a space ?' and (E,dE) is a connected chain Lie algebra of finite type
joined to (L, A1) by a sequence of dgl quasi-isomorphisms. Then the Sullivan
algebras C*(L,dL) and C*(E,dE) are joined by quasi-isomorphisms of commu-
tative dga's and hence suitable application of 'lifting up to homotopy' exhibits
(E, d?) as a Lie model for X.
Similarly, suppose (A, d.4) is a commutative model for X with A° = k, A1 = 0
and each A* of finite dimension. Then the dga quasi-isomorphism C*C(AtdA) ~>
{A,cLa) lifts to a dga quasi-isomorphism C*?(A,dA) ~^ Apl(X), which exhibits
?(A,dA) as a k^e model for X.
This section is organized into the following tOpiCS
324 24 Lie models for topological spaces and CW complexes
(a) Free Lie models of topological spaces.
(b) Homotopy and homology in a Lie model.
(c) Suspensions and wedges of spheres.
(d) Lie models for adjunction spaces.
(e) CW complexes and chain Lie algebras.
(f) Examples.
(g) Lie model of a homotopy fibre.
(a) Free Lie models of topological spaces.
Let X and Y be simply connected topological spaces with rational homology
of finite type. The next proposition establishes the first two bullets in the in-
troduction to this section. Notice however that the 'existence of a CW complex
modelled by a chain Lie algebra', but not the uniqueness is established directly
in §24(d).
Proposition 24.1
(i) The space X has a minimal free Lie model (Lv,d), unique up to isomor-
phism, and any continuous map f : X —> Y has a Lie representative.
(ii) If Ji = Q, any connected chain Lie algebra {L,Al) of finite type is the Lie
model of a simply connected CW complex, unique up to rational homotopy
equivalence.
proof: (i) The construction in §12(a) provides minimal Sullivan models m ? :
(AVx,d) -^ Apl(X) with Vx = {V'?} >0 and each V? finite dimensional,
and ??? : (AVV,d) —> Apl(Y) with the same properties. Apply the Quillen
construction (§23(b)) to obtain natural quasi-isomorphisms
rx : C* (C[/iVx,d)) -^ (AKy.cQ an ?? : C* (C[AVy,d)) ^ (AVY,d) .
Composed with ???, this exhibits ?(Avx,d) and ?(AW,d) as free Lie models of
X and Y. Next, let ? : (i\Vy ,d) —> (AVx,d) be a Sullivan representative for /.
Then ??? = ???*(?^), which exhibits ?? as a Lie representative for /.
Finally, the argument in the proof of Theorem 22.13 now provides a surjective
dgl quasi-isomorphism ? : C^Vx>d) —> (Lv,d) onto a minimal free Lie chain
algebra, which is connected of finite type because C(\vx,d) 1S- This exhibits
(Ly, d) as a minimal free model for X.
Lie Models 325
For uniqueness, suppose m' : C*(Lw,d) —> Apl(X) is an arbitrary minimal
free Lie model. Lift the quasi-isomorphism C*(hy ,d) —> Apl(X) (up to homo-
topy) through m! to a quasi-isomorphism C*(hv,d) —> C*(L\y,d). This yields
a chain of dgl quasi-isomorphisms
(LW,d) 4— Cc(Lw,d) —> ?c-(Lv,d) —> (^V,d) .
Use Proposition 22.11 to invert the first, and Proposition 22.14 to conclude that
the resulting quasi-isomorphism (Ljv,<2) —> 0W,<2) is an isomorphism. The
same argument constructs a dgl morphism between minimal free Lie models
that is a Lie representative for /.
(ii) Apply Theorem 17.10 to obtain
mc-(L,dL) -C*(L,dL) ^APL(\C*(L,dL)\) ,
thereby exhibiting {L,dL.) as a Lie model for \C*(L,dt)\. If C*(L,dL) is a Sul-
livan model for a second simply connected CW complex X then Theorem 17.12
identifies \C*(L,di)\ as a rationalization of ?. ?
(b) Homotopy and homology in a Lie model.
Fix a simply connected topological space X with rational homology of fi-
nite type, choose a Lie model (L,di) for X, and choose a minimal Sullivan
model (AV\,d) for C"{L,di,). We then have specified cochain algebra quasi-
isomorphisms
(AVx,d) -?* C'(L,dL) -2* APL(X)
¦m q
whose composite is a minimal Sullivan model for X.
Now the homotopy Lie algebra L\ is just ?,(???) <8> He with a certain Lie
bracket, and· in Theorem 21.6 we showed that it was naturally isomorphic to
the homotopy Lie algebra of the minimal Sullivan model (AVx,d). Further, in
Proposition 23.3 we used the quasi-isomorphism m to identify this homotopy
Lie algebra with H(L,di). Together these provide an isomorphism of graded
Lie algebras,
aL ¦¦ H(L,dL) ? ?,(??') ® h . B4.2)
As in §21(e) we identify ?»(„?)®!: as the suspension of 7r»(fil)® Jk by writing
sa = —(—l)desad~ia, a G ?»(??) <8> Jk, where <9» is the connecting homomor-
phism for the path space fibration. Then 0l suspends to an isomorphism
tl :sH(L) -^TTm(X)<S>]k .
Now the Lie bracket in ?,(??') <8> ]k, as defined in §21(d), is given by
[?,?] = (-l)de^+1o, [dZla,dZl?]w , ?,? G ??.(??') ,
where [ , ]w denotes the Whitehead product. Since ??, is an isomorphism of Lie
algebras we deduce that "
TLs[a,?] = (-l)dee* [rLsa,TLs?]w , ?,? G H(L) .
326 24 Lie models for topological spaces and CW complexes
Next we observe that a free Lie model (Ly, d) for X also encodes the homol-
ogy of X. Indeed the morphism C*(Ly,d) -^ Api(X) induces a cohomology
isomorphism, which dualizes to an isomorphism i/(C«(Ly,d)) 4^- H*(X;k).
Let d : sV —> sV be the suspension of the linear part dy : V —> V of
the differential d in Ly. Then Proposition 22.8 provides a quasi-isomorphism
C»(Ly .d) -—> (sV ® lc,d). Altogether then we obtain an isomorphism
sH(V,dy) ® k = H*(X;k) . B4.3)
In particular, if (Ly, d) is minimal then Hm(X; Je) = sV ® k.
Finally, [Ly, Ly ] is the ideal in Ly of elements of bracket length at least two.
Thus Ly = V©[Ly,Ly] and [Ly,Ly] is preserved by d, so division by [Ly,Ly]
is a surjective linear map 77 : (Ly,d) —> (V,dv)· As in Example 3, §23(a), we
have
Proposition 24.4 With the hypotheses above the diagram
sH(Lv,d) ~ - ?,(?) <S> Jfc
hurx
sH(V,dv) = H+{X;k)
commutes, and identifies sH{q) with the Hurewicz homomorphism hurx.
proof: The quasi-isomorphism C«(Ly ) —> sV®Jc of Proposition 22.8 converts
the inclusion s(Ly,d) —> C«(Ly) into 577. Thus we have to show that
sH(Lv,d) n.(X)®Je
hurx
HC*(LV) H.(X;k)
commutes. But this is precisely the dual of the commutative diagram at the end
of§13(c). ?
(c) Suspensions and wedges of spheres.
In this topic k = Q. We show that a suspension is, rationally, a wedge of
spheres. More precisely, we establish the
Theorem 24.5 The following conditions on a simply connected topological
space X are equivalent
(i) The rational Hurewicz homomorphism hurx: ?,(?) <8>Q —> H+(X;<Qi) is
surjective.
Lie Models 327
(ii) There is a rational homotopy equivalence of the form V Sna —> X (each
na > 2).
(Hi) There is a well based, path connected space Y and a rational homotopy
equivalence of the form ?? —> X.
(iv) The rational homotopy Lie algebra ?,? is a free graded Lie algebra.
Before proving the theorem we consider the special case that X = V Sn" with
each na > 2, and let ia : Sn<* —> X be the inclusion. The classes [ia] G ??? (?)
are mapped by hurx to a basis of H+(X;Q). Hence they are linearly indepen-
dent and are mapped by the connecting homomorphism to linearly independent
elements wa in ?*(??) <S>z Q = L\. Let W be the linear span of the wa.
Proposition 24.6 With the hypotheses and notation immediately above
(i) kerhurx = s[Lx,Lx}.
(ii) The inclusion of W extends to an isomorphism Lu·· —> Lx.
proof: (i) It is clearly sufficient to prove this for a finite wedge of spheres. But
then Ht(X; Q) has finite type and X is a suspension. Thus (Proposition 13.9) X
has a commutative model of the form k©H with zero differential and ? ? — 0.
Now CikQH is a Lie model for X and clearly has the form Cjk®ti — (Ly ,0). In
particular, Lx = H(hv ) = Ly. Moreover, Proposition 24.4 identifies hurx with
the surjection S7/ : shy —> sV, whose kernel is precisely s[Ly,Ly].
(ii) It follows from (i) that Lx = W ? [Lx,Lx]. Since Lx is free
Proposition 21.4 states precisely that h\v —> Lx. Q
proof of Theorem 24.5: If (i) holds then we can choose based continuous
maps fa : Sn" —> X so that the homology classes H»(fa) [Sna] are a basis
of H+\X;Q). Thus the map / : \/aSna —> X defined by the fa induces an
isomorphism of rational homology. Hence / is a rational homotopy equivalence
(Theorem 8.6) and (i) => (ii).
Clearly (ii) => (iii). Suppose (iii) holds. Use the Cellular models theorem 1.4
to reduce to the case y is a CW complex with a single 0-cell. Moreover, since
L-zY = Lx we may take X = ??.
Now for any finite subcomplex ? C Y we know from Proposition 13.9 that ??
has a commutative model of the form Jk©H with zero differential and ? · ? = 0.
Hence ?ft©/r is a Lie model for ?? and clearly this has the form (L\v, 0). Thus
LZ^H(LW) =LW.
Choose now a graded subspace V C Lx so that V ? [Lx,Lx] = Lx and
extend the inclusion of V to a surjection ? : Ly —> Lx (Proposition 21.4). Let
a e ker ?. Then atlw for SOme finite dimensional subspace W C V. Moreover
328 24 Lie models for topological spaces and CW complexes
by choosing a sufficiently large finite subcomplex ? C Y we can arrange that
W C L-?z and that the morphism L\v —> Lsz sends a to zero. But clearly
W ? [Lt.z,L-?z\ = 0. Thus, since L-zz is free, Proposition 21.4 implies that
Lu-' —> L-?z is injective. Hence ? = 0, and ? is an isomorphism. It follows that
Lx is free; i.e., (iii) => (iv).
It remains to show that (iv) => (i). We show (iv) => (ii), observing that
(i) is a trivial consequence of (ii). If Lx = Lv choose based continuous maps
fa : Sn" —> X such that the homotopy classes [fa] G ???(?) are mapped by
the connecting homomorphism d, to a basis va of V C ?? (= 7?,(??") ®Q).
The fa define a continuous map / : \JaSn° —> X.
On the other hand, write S = \/aSna and let ia ¦ Sn° —> S be the inclusion.
Then by Proposition 24.6, the elements wa = d.[ia] are the basis for a subspace
W C Ls such that Ls = Lw. Since 7?.(?/) : wa ?—> va it follows that 7?*(?/) ®
Q is an isomorphism and / is a rational homotopy equivalence. ?
Example (Baues [19]) If H+(X;Q} is concentrated in odd degrees then X has
the rational homotopy type of a wedge of spheres.
Here we consider simply connected spaces A' with rational homotopy type of
finite type. Let (Ly, d) be a rational minimal Lie model for X (Proposition 24.1)
then d restricts to zero in V and so sV - H+(X;Q), by B4.3). Since H+(X;Q)
is concentrated in odd degrees, V = Veven and thus Ly is concentrated in even
degrees too. For degree reasons, then, the differential in Ly is zero. Now the Lie
algebra isomorphism B4.2) shows that ?,(??') <g)Q is a free graded Lie algebra,
and the conclusion follows from Theorem 24.5. ?
(d) Lie models for adjunction spaces.
Consider an adjunction space
where:
(i) X is simply connected with rational homology of finite type,
(ii) f={fa:(Sn",*) -> (A>0)}and
(iii) the cells Dn° + l are all of dimension > 2, with finitely many in any given
dimension.
Suppose that
m : C*(Lv,d) —> APL(X)
is a free Lie model for ?\ We shall construct a free Lie model for Y. In fact, in
§24(b) we constructed the isomorphism
tl : sH(Lv) ? ?»(?) <g> k
Lie Models 329
Thus the classes [fa] G ???(?) determine classes s[za] = T~l[fa] e sH(Lv)
represented by cycles za G Lv- Let IV be a graded vector space with basis {wa}
and degwa = na. Then we can extend L\/ to a chain Lie algebra Lv$h·' =
L(V © W) by defining dwa = za.
Theorem 24.7 The chain Lie algebra (hv@w,d) is a Lie model for Y.
proof: Decompose C*(Ly,<2) as the tensor product of a minimal Sullivan al-
gebra (AVx,d) and a contractible Sullivan algebra. This exhibits (AVx,d) as a
minimal Sullivan model for A". Let U be a graded vector space with basis {ua}
and degua = na + 1. Then it is shown in Proposition 13.12 that a commutative
model .4 = (AVX © U, dA) for Y is given by setting:
• AVx is a sub algebra.
• U'A+ =0, and dA(U) =0.
• dAv = dv + ?(?; [fa])ua , ? G Vx ,
a
where we have already identified ?*(?') <S> Jk with Hom(Vx,]k) as in Theo-
rem 15.11. (In fact, the proof in 13.12 is for a single cell, so that there U = 3cu.
but the argument in the general case is identical.)
We proceed now in four steps.
Step (i): Description of Ca ¦
Let (C,dc) be the dgc dual to (AVx,d), so that C(\vx,d) = {Hs~lQ,dc)-
Denote Hom(i7, Je) by U*, with dual basis u*a given by {u$,u*a) = ???. Then the
graded coalgebra dual to .4 is just C ®U*, and the elements of U* are primitive:
Ku*a = 0- The differential, ?, in Hom(.4, A·) reduces to dc in C and satisfies
5u*a = (— l)des3iQcQ, where ca G C is the primitive cycle given by
(v,ca) = (v;[fa}) and (A*2Vx,ca) = 0 .
Thus CA = (Hs-^Qs-^^^a) with dA restricting to dc in L(s-1C) and
Step (it): Identification of the za.
The cycles ca G C = Hom(AVjv, A·) restrict to the linear functions ca G
Hom(Vx,k) which we have identified with [fa] G ??? (?) ® Jk. On the other
hand, the decomposition of C*(Ly,d) as (AVx,d) <g> E. with ? contractible,
defines a surjective dga quasi-isomorphism
which is left inverse to the inclusion. The linear part. Qo, of ? therefore dualizes
to an inclusion,
?0 : (Eom(Vx,k),0) —» s(Lv,d) ,
330 24 Lie models for topological spaces and CW complexes
and ?(??) is inverse to the isomorphism r. Thus T~l[fa] = H(uo)(ca) = [oocq]
and we may take
Step (iii): . The dgl quasi-isomorphism CA —> (L(^ ? W),d).
The quasi-isomorphism ? of Step (ii) dualizes to a dgc quasi-isomorphism
? : (C,dc) —> C*(Ly,d) and hence defines the dgl quasi-isomorph'ism
? : C(C,dc) -^H ?(C.(W)) A (Lv,d) ,
where ? is the quasi-isomorphism of Theorem 22.9. Moreover C(C,dc) =
L{s~1O) and ?C~1??) = s~1luoc0i = za, as follows from the definition of -?.
Extend ? to a morphism of graded Lie algebras
by requiring ??^ = (~l)desCawa. By Step (i), ? a is a dgl morphism from
?.4 to (L(F Q\V),d), as defined before the statement of the theorem. Since
? is a quasi-isomorphism, so is its linear part: s~xC —> V, as we showed in
Proposition 22.12. By construction, the linear part of ?4 sends s~lU* isomor-
phically to W. Hence the linear part of ?,4 is a quasi-isomorphism, and a sec-
ond application of Proposition 22.12 establishes ?,4 as a dgl quasi-isomorphism,
Step (iv): (L(V ? W),d) is a Lie model for Y.
Since (A, dA) is a commutative model for Y the quasi-isomorphism C*(CA) -^>
(A, d) identifies C*(?A) as a Sullivan model for Y and (?A,d) as a Lie model
for y. Since (?A,d) ~ (L(V ? W),d) the latter is a Lie model for Y as well. ?
Remark In the setup at the start of §24(d) let
j : X —*· Y
denote the inclusion. It follows from the Remark after Proposition 13.12 that
the surjection (A,dA) —> (AVx,d) is connected by a chain of commutative dga
quasi-isomorphisms to Apl(j). A tedious chase through the proof of Theo-
rem 24.7 will therefore establish a homotopy commutative diagram
C*(Lv) « C*(A) C-
APL(X) . APL(Y) ,
where the left hand vertical arrow is the model with which we started and ?
(Lv,d) —> (LvQ\v,d) is the inclusion.
Lie Models 331
(e) CW complexes and chain Lie algebras.
Suppose X is a connected CW complex with no 1-cells and finitely many cells
in each dimension. Then we can use Theorem 24.7 to construct a Lie model
(Ly, d) for X such that: 'each (Ly<n , d) is a Lie model for Xn, a basis {va} of Vn
corresponds to the (n + l)-cells D^+l of X, and s[dva] G sH(Lv<n ) corresponds
to the class [fa] of the attaching map fa : S? —> Xn under the isomorphism
tl : sH (Lv<n ) ? ??,(???) ® Q of §24(b).
We say that (Ly, d) is a cellular Lie model for X.
Conversely, let (Ly ,d) be a connected free chain Lie algebra of finite type
defined over Q. Then we can use Theorem 24-7 to construct a CW complex X
for which (Lv,d) is a cellular Lie model.
Indeed, suppose the ?-skeleton, Xn is constructed and (Ly<n_,, d) is identified
as a Lie model for Xn. Then the isomorphism 77, identifies
Choose a basis {va} of Vn so the classes s[dva] correspond to elements in irn(Xn);
i.e. are represented by continuous maps fa : Sn —> Xn, and set
Xn+1 = XnU{fa}
Now Theorem 24.7 identifies (Ly<n ,d) as a Lie model for Xn+\.
This provides an inductive construction of X. By the Remark following The-
orem 24.7 there is a homotopy commutative diagram of commutative dga's,
- - - - C (Ly<n , d) ' C"{X) C* (Ly<„ , d) - - -
I I
-\ \-
¦¦¦ - APL(Xn) APL(Xn+1) -
Since C*(Ly,d) —> C* (Ly<n ,d) is an isomorphism in degrees < ? + 1, it is
straightforward to construct a quasi-isomorphism C*(Ly ,d) ^* Apl(X). Thus
(Ly,d) is a cellular model for X.
(f) Examples.
Example 1 (Ly, 0) is the minimal Lie model of a wedge of spheres.
In fact Theorem 24.7 asserts that for any V = {Vi}{>1 of finite type with basis
RJ, (Ly,0) is a Lie model of the space X = \JaSn°+1 = pt\Jf ( \J Dn°+1 ),
V Of /
deguQ = na. Note that this also follows from §24(c). ?
Example 2 The free product of Lie models is a Lie model for a wedge, \JaXa.
Suppose Xa are simply connected spaces and X = \JaXa has rational homol-
ogy of finite type. Let (LV(a), da) be a Lie model for Xa.
332 24 Lie models for topological spaces and CW complexes
Recall the free product defined in §21(c) and note that \J (Ly(a),da) is
the free dgl L^^.d , in which d restricts to da in each V(a). Simplify
the notation (LV(a),da) to (La,da)- Then C*(La,da) is a Sullivan model for
Xa. Now the Example of §12(c) exhibits the fibre product ? (C*(La,da)) as
a Ik
a commutative model for X. On the other hand the inclusions (La,da) —>
{J (La, da) induce a dga morphism
Of
C*(u (?*> da)) -> ? ik (C*(La, da)) -
V or Ja
We show that this is a quasi-isomorphism, thereby exhibiting JJ (La,da) as a
Q
Lie model for \/aXa.
To see that this is a quasi-isomorphism note that it is the dual of the horizontal
arrow in
in which the slant arrows are the quasi-isomorphisms of Proposition 22.8.
Finally, observe that the homology of a free product is the free product of the
homologies, so that
?. (?\/???) ® Q = h(u U'(a),da) = U ??*?) ·
\ ? J a
Example 3 The direct sum of Lie models is a Lie model of the product.
Again let (La,da) be Lie models for simply connected spaces Xa such that
X = Y\ Xa has rational homology of finite type. Then only finitely many Xa
a
will have rational homology degrees less than any given ? and so we may suppose
only finitely many La have elements in degrees < n. It follows that
C* (g)(La,da)) =CaC*(La,da) ..
Lie Models 333
which exhibits 0(?a,da) as a Lie model for X (Example 2, §12(a)). ?
Of
Example 4 A Lie model for S3 V S3 U(ct>(ct^]iv]vv. D8.
Let ?,? G ti3(S3 V S3) be the elements represented by S3 and S3 respectively.
Then (cf. Example 1) a Lie model for S3 V S3 is just (L(v,w),Q) with degu =
degw = 2 and u,u> corresponding to ?,?. Moreover, the isomorphism 7i\,(S3 V
S3) ®Q s sL(u, u>) identifies [a, [a, ?]w]w with 5 [?. [?, ??]], as is shown in §24(b).
Hence by Theorem 24.7,
(L(u,u;)u).du = [u[u,u>]]) is ? Lie model for S3 V S3. U[Q>[QKj,r] D8.
?
Example 5 Lie models for CP°° and CPn.
The space CP°° is a K(Z, 2) and so its minimal Sullivan model is A = (?(?). 0)
with dega = 2 — cf. §15(b), Example 2. Thus CA is a Lie model for CP00.
This Lie model has the form L(i»i ,??,??, ¦ ¦ ¦ ) with ?,· the desuspended dual of
a!. (Thus degvi = 2i — 1). In the coalgebra C dual to .4 let c; be the element
dual to a*. Then Ac^ = ^Z ci ® ci· Thus the formula in §22(e) shows that the
i+j=k
differential in CA is given by
dVk = 2 2^ ^'^J ·
Since H(CA,d) = 7r»(CP°°) ® Q it follows that
, ? = 2
Hn(CA,d) =
0 , otherwise,
a fact that may not be immediately obvious from the formula for d.
Notice that the construction of a CW-complex for this Lie model (§24(e)) has
one cell in each even degree 2n and no cells of odd degree. The 2n-skeleton has
the rational homotopy type of CPn, with Lie model C(v\,... ,vn) and the same
differential.
In this model ? [vhvj] is a cycle whose suspended homology class in
i+j=n+l
7T2n+i (CPn) ® Q is not a Whitehead product. ?
Example 6 A Lie model for (CP2 V S3) U(Q)?]H, D8.
As we saw in Example 5·, a Lie model for CP2 is just (L(ux ,vo),d) with dvo =
|[ui,ui]. Thus [v\,vo\ is a cycle and the homology class of s[ui,uo] corresponds
to a class a G tt5(CP2) <g> Q. Let ? G ^(S3) be the fundamental class. Then as
in Example 4, Theorem 24.7 shows that
,v-2,w,u); dvo = -[vi,v2}, dw - 0, du =
334 24 Lie models for topological spaces and CW complexes
is a Lie model for (CP'2 V S3) U[a,?]w D8. ?
Example 7 Coformal spaces.
A simply connected topological space X with rational homology of finite type
is called coformal (sometimes ?-formal in the literature) if it has a Lie model
(L, 0) with zero differential. In this case L is the homotopy Lie algebra Lx
(§21(d), (e)); i.e., the rational homotopy type of X can be formally deduced from
its homotopy Lie algebra. (Note the parallel with formal spaces as defined in
§12(c).)
If X is coformal with Lie model (L, 0) then C*(L, 0) is a Sullivan model for X
of the form (AVx,di) with d\ : ?? —> ?.2\'?. Conversely any Sullivan model of
this form can be written as C*(L, 0) — cf. §23(a): X is coformal if and only if
it has a 'purely quadratic ' minimal Sullivan model.
Finally, recall from Example 1 in §23(a) that H(C*(L,0)) = ExtUL(lk,lk).
But ULX = H*(nX;]h), as we saw in Theorem 21.5. Thus
X coformal => H*(X; k) = ExtH^Qx.k) (k, k). ?
Example 8 Minimal free chain Lie algebras.
Suppose (Lv, d) is a minimal connected free chain Lie algebra of finite type,
defined over Q and let X be a CW complex for which (Ly ,d) is a cellular Lie
model (§24(e)). Then
• sV ? Q = H*(X; Q), by the last comment in §24(b).
• A basis of V is in 1-1 correspondence with the cells of positive dimension
of X, which also form a basis of the reduced cellular chain complex of X.
The conclusion is that the differential in the rational cellular chain complex of
X must be zero, and hence the differential in the integral cellular chain complex
is zero too. In other words:
H*(X;Z) is a free Z-module with basis corresponding to a basis of Q® sV. ?
(g) Lie model for a homotopy fibre.
Let
0 —»¦ (/, d7) —»¦ (L, dL) A> (A-, dK) —». 0
be a short exact sequence of differential graded Lie algebras (connected and of
finite type). By taking cochain algebras (see Section 23(a)), we obtain a relative
Sullivan algebra (see Section 14)
C*{K,dK) ^4 C*(L,dL) -^C*(I,dj) .
Proposition 24.8 When ? is a Lie representative for a continuous map f :
X —> Y between simply connected spaces, then (I, dj) is a Lie model for the
homotopy fibre of f.
Lie Models 335
proof: By hypothesis there is a homotopy commutative diagram
C'(K,dK) ^ APL(Y)
C'(p)
C*(L,dL) 22—+ APL{X) .
B4.9)
Now let H be the homotopy from ???€*(?) to ApL{f)my. Then in the com-
mutative diagram
C*{K,dK) —2— APL(X)®A(t,dt)
C'(p)
C*{L,dL)
we may lift ?? ? through id ®?o because C*{p) is the inclusion of a relative Sulli-
van algebra (Proposition 14.4). This provides a homotopy ??? ~ m'x such that
m'xC*(p) = ApL(f)my. In other words we may suppose that B4.9) commutes
exactly.
Now Proposition 15.5 identifies C*(I, dj) as a Sullivan model for the homotopy
fibre, F, of /, and hence by definition identifies (/, dj) as a Lie model for F. ?
Exercises
1. Determine the minimal Lie models of the spaces:
CP"#CP" , S3 x S3 x S3 , CP4/S2 , CP5/S'2 .
2. Show that if X is a simply connected CW complex and Ht(flX; Q) is a tensor
algebra, then X has the rational homotopy type of a wedge of spheres.
3. Let (AV, d) -> (A, 0) be a Sullivan minimal model with V1 = 0, and let ?
be of even degree and ? : (A <g> ??,?) —>· ? = (A ® Ax/1,0) a projection whose
kernel is the ideal / C A <S> A+x.
a) Prove that there is a quasi-isomorphism (A®Z, 0) —>· (^<8>?(x) ??, D) where
? = (kerd ? ?) <S> Ax.
b) Suppose that the continuous map f : X -*¦ Y admits ? as commutative
model, and let F be the homotopy fibre of /. Prove that F admits a model of
the form (.4 © ?) ?(??,?) E(V) and thus that F has the rational homotopy type
of a wedge of spheres.
c) Prove that there exists a short exact sequence of graded Lie algebras
0 _> L(V) -> ?,(??-) ? q ?'^/] ?,(??) ® 0 -> 0.
336 24 Lie models for topological spaces and CW complexes
4. Let H — A(xi,... ;Xn)/I, where the rr;'s have even degree and where the ideal
/ is generated by monomials. Prove that H = ((··· {/\X\)/I\ <g> ??2)/-^2 ® - · - ®
Axn)/In , where each Ip is an ideal in A{xi,X2,- ¦ ¦ ,xp) contained in the ideal
generated by xp. Let X be a formal space such that H*(X;Ql·} = H. Deduce
from exercise 3 that there is a finite sequence of extensions
0-»L(Vi) ->?i -» (??,... ,xn) ->0
0 ->L(V2) -> L-2 ->Li -> 0
0 -» L(Fn) -> ?.(??') ® Q -> Ln_! -» 0 s
where (xi,... , a;n) denotes the abelian Lie algebra on the variables ? ?,??,- ¦ ¦ :xn-
5. Let X and y be simply connected CW complexes of finite type. Prove that
Lxvy — LxlJLy. Suppose that ? G (Lx)even and y G (Ly)even. Prove that
L(x,y) C LXvy.
25 Chain Lie algebras and topological groups
In this section the ground ring is Q.
In this section we pass from algebra to CW complexes using the spatial realiza-
tion functor | | of §17(c). It will often be convenient to abbreviate the notation
\A,d\ simply to \A\. We shall also abbreviate \C*(L,cLl)\ simply to \C"L\.
Let {L,cLl) be an arbitrary connected chain Lie algebra of finite type. Our
principal objective is to
• Convert the universal enveloping algebra {UL,di) to a topological group
\TL\.
• Construct a contractible CW complex \C*(L;FL)\ and a principal fibre bun-
\C*(L;FL)\ -^ \C*L\ , \C*{L;TL)\ x \TL\ -> \C*{L;TL)\ ,
thereby identifying \C*L\ as a classifying space for \TL\.
In particular, if (L, ci/,) is a Lie model for a simply connected topological space
X then we will deduce a chain of rational equivalences of topological monoids
from ?? to |??|.
This section is divided into the following topics
(a) The topological group \TL\.
(b) The principal fibre bundle \C*{L;TL)\ A- \C*L\.
(c) \TL\ as a model for the topological monoid, ?.?.
(d) Morphisms of chain Lie algebras and the holonomy action.
Throughout we shall work with the spatial realization functor (§17(c)) from
commutative cochain algebras to CW complexes. We recall that it converts the
tensor product of cochain algebras to the topological product of spaces (Example
of§17(c)).
(a) The topological group, \TL\.
Recall that the universal enveloping algebra, (UL, d) is a dg Hopf algebra (§21)
which is cocommutative as a coalgebra. Thus its dual (TL, ??) = Horn {UL, Q) is
also a dg Hopf algebra with commutative multiplication ?? and comultiplication
Af dual, respectively, to the comultiplication and multiplication in UL. Since
TL is commutative, both ?? and ?? are dga morphisms.
Proposition 25.1 The CW complex \TL\ is a topological group, with multipli-
cation |??| and topological diagonal \??\.
338 25 Chain Lie algebras and topological groups
proof: The coassociativity of ?? implies that
|??| : \TL\ ? |??| -> |FL|
is an associative product. The single 0-cell of |FL| is the augmentation e : TL —>
Jc, and its compatibility with ?? implies that e G |FL| is a two-sided identity.
To construct an inverse we first construct a distinguished involution ? in UL.
Let ULopp be the dg Hopf algebra defined as follows: as a differential graded
coalgebra, ULopp = UL. However the product in ULopp is given by a-b =
(_l)dega deg6ouj where ba is the product in UL. Define a : L —*· ULopp by
ax = —x. Then a[z,?/] = ax-ay — (— l)desx de%yay -ax, and so a extends to a
dga morphism v.UL—y ULopp.
We may regard ? as a self morphism of the dgc, UL. Clearly v2 = id, and so
? is an automorphism satisfying v(ab) = (—l)desa ae%bba.
Observe next that the composite
coincides with UL -^> k -^—y UL. In fact, if ? G L then ?? = mult(a; ® 1 —
1 <g> ?) = 0. Moreover if ?? = ??* ® a[ and ?6 = ?bj <S> b'j then
?{(?) = 22l±aibj{vb'j)va'i = Y^±ai((pb)i/a'i .
Hence if <pb = 0 then <p(ab) = 0 and so ? = 0 in I7L+.
Let v* = Hom(f,wfe) be the dual of v. It is an automorphism of the cochain
algebra (FL,Si). Apply | | to the dual of the formula above for ? to see that
\i/*\ is an inverse in |FL|, which is therefore a topological group.
Finally, note that in any commutative cochain algebra (A, d) the multiplication
?A realizes to the topological diagonal in \A,d\, as follows immediately from the
definitions. ?
(b) The principal fibre bundle, \C*(L;FL)\ A- \C*L\.
Recall the acyclic construction C*(L; UL) considered in §22(c). It is, in partic-
ular, a cocommutative differential graded coalgebra of the form (A.sL <g> UL, d).
Moreover, as we remarked in §22(c), the projection ? : (AsL<S)UL,d) —> (AsL, d)
and the right module action a : (AsL <g> UL, d) <g> (UL, d) —> (AsL <g> UL, d) are
both dgc morphisms.
Apply the realization functor to the cochain algebra C*(L; TL) dual to (AsL®
UL,d), to construct the CW complex |C*(L,-FL)|. (Note that the differential
in C*(L;TL) = (C*(L) (&TL,d) is not the tensor product differential, and so
|C*(L;FL)| is not the product of \C*L\ and |FL|!). The duals q* and a* of the
morphisms above realize to continuous.maps
\C*(L;TL)\ ^h \C*L\ and \C*(L;TL)\ ? \TL\ —*· \C*(L;TL)\ .
Lie Models 339
Proposition 25.2 The construction above is a principal \FL\-fibre bundle
whose total space. |C*(L,-FL)|, is a contractible CW complex.
proof:
Step 1: C*(L) is a Sullivan algebra and the inclusion ofC*(L) in C*(L;YL) is
a relative Sullivan algebra.
The first assertion is Lemma 23.1. For the second recall the isomorphism
7 : AL -^ UL of Proposition 21.2. It is obvious that 7 preserves differentials and
the comultiplication. Thus, dually, (FL,??) = {AL*,—d*L) as graded algebras,
L* denoting Hom(L, <Q) and d*L the dual of cLl- It now follows exactly as in
Proposition 23.1 that C*{L;TL) = (C*(L) <8>AL*,d) is a relative Sullivan algebra
with respect to the inclusion of C*(L).
Step 2: \C*(L;FL)\ is a contractible CW complex.
By Step 1, C*{L;TL) is itself a Sullivan algebra of the form (AV,d) with
V = (sL <g> 1)* 6A® L)*. The linear part of the differential, do : V —*· V, is
dual to the restriction of the differential in C,(L; UL) to sL ® 1 ? 1 <g> L. Since
d(sx <S> 1) = -sdLx <g> 1 + (-l)de6*+ii ® x and d{\ ® x) = 1 ® d/,? it follows
that (sL ® 1) ? A <g> L) = (sL ® 1) © rf(sL ® 1). Dually, ? = U ? cfei/ with
d0 : U A doi7. From §14(b) we deduce that C{L;TL) = A(U ® dU) with
d : U A dC/.
Now let {ua} be a basis of U. A dga morphism ? : A(U ? dU) —> (Apl)u is
specified by the arbitrary choice of elements tpua G (ApL)„esUa. This identifies
(A(U@dU)) as the extendable simplicial set UApll- Thus \C*{L;TL)\ = \A{U®
a
dU)\ is a contractible CW complex (Example 3, §17(a)).
Step 3: pL : |C*(L,-FL)| —)· \C*L\ is a principal \TL\-fibre bundle.
Recall that C"(L;TL) = (C*(L) ®TL,d). An ?-simplex of \C'L\ is a dga
morphism ? : C*(L) —> (Api,)n. As in the proof of Proposition 17.9, the
pullback of pl over ? has the form
?" X](Apc)n\ \{APL)n ®C*(L) (C*(L) ® TL, d) I .
If we can exhibit this as ?? ? |FL|, compatibly with the projection on ?" and
the action of |FL|, then Proposition 2.8 will apply and show that pi is a principal
bundle.
Denote {APL)n by A and (APL)n ®C-(L) {C*{L)®TL,d) by (A <g> TL,d).
Note that the dual, a*, of the Z7L-action a induces an obvious morphism ? :
(A <g> TL,d) -+ (A® TL,d) ® (FL,6L). Since ? {A) = Q, the argument of
Lemma 14.8 with Q —> A replacing B-i —> ? shows that id a extends to an
isomorphism V : (A <&FL,d) -=> (A, d) ® (FL,6l)- Combine this with the
augmentation, e, of (TL, 6L) to define {??®?)? : {A®TL,d) —)· (A,d). The
composite
<p:{A® TL, d)A(A® ??, d) ® {TL, ol) i!^!^!i (A, j) 0 (??> Sl)
340
25 Chain Lie algebras and topological groups
reduces to the identity in .4 and satisfies ?A<?>?) — 1<?>? € ?+®??,, ? G TL, Hence
? is an isomorphism. It clearly converts ? into id ®??- Thus \?\ : |.4| ? |FL| -=»
|,4 ®FL.d\ compatibly with the projection on |.4| and the action of |??|. ?
Notice that Proposition 25.2 identifies \C*L\ as weakly homotopy equivalent
to the classifying space (§2(e)), B^l\- In fact (cf. §2(e)) the principal bundle
? : \C*(L;TL)\ —> \C*L\ pulls back from the universal bundle E^l[ via a map
/ : \C'L\ —> B{rL{. Since it, (\C*(L;TL)\) = 0 = ??. (E[rL\), / is a weak
homotopy equivalence.
(c) \TL\ as a model for the topological monoid, ?.?.
Suppose now that (L,dL) is a Lie model for a simply connected CW complex.
X. In particular we have a dga quasi-isomorphism
which in turn (Theorem 17.12) defines a rationalization ?? : X —> \C*L\. Thus
?/?.? : ?? —> Q\C*L\ is a morphism of topological monoids and also induces an
isomorphism of rational homotopy and homology (Theorem 8.6). We call such
a morphism a rational monoid equivalence.
On the other hand suppose G is any topological group and ? -^> ? is any
principal G-bundle with 7r,(Z) = 0. Then from Proposition 2.10 we obtain weak
equivalences of topological monoids
GP-GxzPZAfi5, a<—i(a,u/)i—>p°w.
and corresponding weak equivalences of Serre fibrations
PZ
PB
Thus here we have the chain of rational monoid equivalences
|??| <=- |??| X|c-(L;rL)i P\C*{L-TL)\ A il\C"L\ ?^
B5.3)
thereby exhibiting |FL| as a 'topological model' for ??'". We also have the
corresponding (rational) equivalences of Serre fibrations
\C'(L\rL)\
P\C'(L;rL)\
\C'L\
B5.4)
Lie Models 341
(d) Morphisms of chain Lie algebras and the holonomy action.
Suppose ? : (L, di) —>· (E, d?) is a morphism of connected chain Lie algebras
of finite type. Then U<p : (UL,dL) —> (UE,d?) is a morphism of dg Hopf
algebras. This is, in particular, a representation of {L,di) in (UE,d?), and
therefore determines the chain complex (C*(L;UE),d) as described in §22(b).
This example has many of the additional properties of C*(L; UL). It, too, is a
cocommutative differential graded coalgebra and a right (UE,d?)-module, with
the module action a dgc morphism. Moreover, C»(</>) : Cm(L,di) —> ?*(?,??)
extends to the morphism of dgc's and ?7i?-modules:
C.(</>; id) : C.(?; UE) —> C.(E; UE) .
Now apply the spatial realization functor to the commutative cochain algebras
(and morphisms of same) dual to these constructions. This gives the commuta-
tive diagram of continuous maps
\C*(L;FE)\ |C*(y;id)| - \C*{E;TE)\
\C*L\ _ . \C'E\
in which: ? is a principal |F?7J—fibre bundle (exactly as in Proposition 25.2)
and \?*(?; id)\ is a map of |r\E|-bundles. In particular, this exhibits ? as the
pullback of pe via |C"V|-
On the other hand, the continuous map \C*ip\ determines a holonomy fibra-
tion as described in §2(c), which is just the pullback via \?*?\ of the Q\C*E\
fibration P\CE\ —> \CE\. We may therefore apply Proposition 2.11 to obtain
equivariant weak equivalences of Serre fibrations
\C*(L;rE)\ = ? -Z-~ \C*L\ X|C-E| P\C'E\
, |??| ?- ? ^ U\C*E\
\C'L\
B5.5)
connecting the principal \TE\-fibre bundle ? with the holonomy fibration for
\cy\.
Finally, suppose / : X —> Y is a continuous map between simply connected
CW complexes with rational homology of finite type. Assume further that
(L,di,) and (?,??) are Lie models for Ar and for Y and that ? : (L,di) —>
342
25 Chain Lie algebras and topological groups
makes the diagram
APL(Y)
APL(X)
B5.6)
C\E,dE)
?'(?)
C*(L,dL)
homotopy commute in the sense of §12(b). In analogy with §25(c) we observe
that the principal \TE\-fibre bundle ? : \C*(L;TE)\ —> \C*L\ is equivariantly
rationally equivalent to the holonomy fibration ? ? ? PY —> ?.
In fact, the diagram B5.6) produces the homotopy commutative diagram
X
Y
hx
hY
\C*L\
\C*E\
(cf. §17(d)) in which hx and hy are rationalizations. Regard the homotopy as
a map ? from X to the space of Moore paths in \C*E\ of length 1, such that
(??)@) = \?*?\???(?) and (??)A) = hyf(x). Then an equivariant rational
equivalence
h:XxYPY-+\CmL\x]C'E\P\CmE\
B5.7)
is given by h(x,w) — (???,?(?) *hy o»). Combined with B?.?) above this
provides the desired equivariant rational equivalence. ?
26 The dg Hopf algebra Cm(QX)
In this section the ground ring is Q; as usual we simplify notation by writing
C.(-) and H.(-) for C.(-;Q) and H.(—,Q).
Suppose given a free Lie model (Ly,d) for a simply connected topological
space X with rational homology of finite type. A natural isomorphism of graded
Hopf algebras,
H(ULv,d)^H,(ClX) B6.1)
is then described as follows. First, H{Uhv) = UH(LV), by Theorem 21.7.
Next, H(Ly) is identified with the homotopy Lie algebra L\ by B4.2). Finally,
the graded Hopf algebras ULx and ?*(??) are identified by the Milnor-Moore
theorem 21.5.
The main objective of this section is to show (Theorem 26.5) that the isomor-
phism B6.1) is induced from a chain algebra quasi-isomorphism
Q:(ULv,d) -=>C.(ilA")
which commutes with the comultiplications up to dga homotopy (to be defined in
§26(a)). This is a result of Majewski [119]. Its significance is due to a theorem of
Anick [11] who showed directly the existence of a unique quasi-isomorphism class
of free chain Lie algebras (L, d) admitting such a quasi-isomorphism. However,
these were potentially different from the Lie models constructed via Sullivan's
functor Apl as described here. Majewski's result shows that they coincide.
Our proof of Majewski''s theorem is different from his, which takes a more
abstract approach (permitting him to prove that other forms of introducing Lie
models also produce the same answer). The idea of the proof here is as follows.
In §25(c) we connected UX to a certain topological group, |FLv|, by rational
monoid equivalences. This'reduces the problem to constructing an appropriate
quasi-isomorphism (ULv,d) -=» C,|rLy|. For this we use the integration map
/.of§17(f).
Finally, at the end of this section, we consider continuous maps / : X —> Y
and prove an analogous theorem about the holonomy fibration.
This section is divided into the following topics:
(a) Dga homotopy.
(b) The dg Hopf algebra C*(QX) and the statement of the theorem.
(c) The chain algebra quasi-isomorphism ? : (t/Ly,d) -=> C^IXy,^).
(d) The proof of Theorem 26.5.
In carrying out these steps we shall make frequent use of the Alexander-
Whitney and Eilenberg-Ziller chain equivalences
(-) and EZ : <?»^
344 26 The dg Hopf algebra C.{ilX)
defined in §4(b) for topological spaces. As observed in §17(f), these equivalences
are defined for all simplicial sets, and we shall use them as such. In particular
we shall quote the important properties D.4)-D.10) as if they had been stated
for simplicial sets, since they hold in thus wider context.
(a) Dga homotopy.
Suppose given a chain algebra of the form (TV, d). Given two graded algebra
morphisms ?,?' : TV —> A we say a linear map ? : TV —> A is a (?,?1)-
derivation if
<&(xy) = ?? Vy + (-l)degxdeg<IV:z;-3>y , x,yeTV .
Any linear map V —> A extends uniquely to a (?, <?>')-derivation.
Two chain algebra morphisms ?. ?' : (TV,d) —> (A,d) are dga homotopic if
? — ?' = ?? + ??
for some (?, ?')-derivation ?, in which case we write ? ~ ?'. The derivation ? is
called a dga homotopy. Note that both sides of such an equation are themselves
(?, <//)-derivations. Thus in constructing a dga homotopy it is sufficient to verify
that this equation holds in V.
Dga homotopy appears to be quite distinct from Sullivan's homotopy described
in §12(b). In fact the two are closely related, but we shall not pursue this here.
The following lifting lemma parallels all the others.
Lemma 26.2 Suppose ? : (?, d) -=> (.4, d) is a chain algebra quasi-isomorphism
Then
(i) For any chain algebra morphism ? : (TV, d) —> (A, d) there is a morphism
? : (TV,d) —> (B,d) such that ? ~ ??.
(ii) If ?,?' : (TV,d) —> (B,d) are morphisms satisfying ?? ~ ??' then ? ~
?'.
proof: (i) We construct ? and a homotopy ? : ? ~ ?? by induction. Suppose
they are defined in T(V<n) and choose a basis {va} for Vn. Then the cycles
???? satisfy ?(????) = ???? — ????? = ?(??? — ????). Since 77 is a quasi-
isomorphism there are elements ba G ? and aa G A such that dba = ???? and
?? = ???? — ???? + daa. Extend ? and ? to Vn by setting ??? = ba and
??? — uq- Then ? extends uniquely to an algebra morphism from TV<n and
?? = ?? because this relation holds in V<n. Similar ? extends uniquely to a
(?, rcT/O-derivation from TV<n to ? and ? — ?? = ?? + ?? because this relation
holds in V<n-
(ii) By hypothesis there is an (??, ??')-derivation ? such that ?? —
??' = B?+?B, and we seek to construct a (?, VO-derivation ? such that ?—?' =
d* + *i!d. Assume ? constructed in V<n so that ?? — ? = dE — Ed for some
Lie Models 345
(??, 77^')-derivation ?. Again let {va} be a basis of Vn. Then (?-?')?? -
is a cycle za in B, and ??? = ???? - (?^ - ?)??? = ?(??? - Edva). Choose
ba G 5 and aa ? A so that zQ = <2bQ and ?? = ??? - ?B?? + daa. Then extend
? and ? by setting #uQ = ba and ??? = aa. D
Lemma 26.3 5uj9j[?ose ? : (TW,d)
morphisms of chain algebras.
(i) Ififo ~ ?? then ?(?0) = ? (??),
(ii) Ififo ~ ?? then ??0 ~ ??! and ??? ~ ?\?.
(iii) Dga homotopy is an equivalence relation.
proof: (i) This is immediate from the equation ?? — ?\ = ?? + ??.
(ii) If ? is a dga homotopy from ?? to ?? .then ?? is a dga homotopy
from ??? to ??? and ?? is a dga homotopy from ??? to ?\?.
(iii) Reflexivity: The zero map is a dga homotopy from ?0 to ?0.
Symmetry: First construct a new dga (/, d) as follows. Let V and
F" be graded vector spaces isomorphic with V and let sV be the suspension
of V with suspension isomorphism ? ?—> sv. Put / = T(V ? F" ? sV) and
let i', i" : TV —> I be the algebra inclusions extending the linear isomorphisms
V s V',V". Let 5 : TV —->· / be the (i',i")-derivation determined by 5u = su.
Finally, let d be the derivation of / determined by di'v = i'dv, di"v = i"dv and
dsv = i'v — i"v — Sdv, ? ? V.
Next, verify in sequence the equations di' = i'd, di" = i"d, i' — i" = dS + Sd
and d2 = 0, noting that it is sufficient to check each on the appropriate generating
subspace. In particular (/, d) is a chain algebra, and i' and i" are chain algebra
morphisms.
Now suppose ? is a dga homotopy from ?? to ?\. Define a dga morphism
? : (I,d) —>¦ (A,d) by ?,?' = ?0, ?" = ?? and Clsv = ??. We show that i" ~ I
and conclude that ?? = Qi" ~ Qi' = ??·
For this define a dga morphism ? : (I, d) —> (TV, d) by ??' = id = ??" and
??? = 0. If i' is a quasi-isomorphism then so is ? and hence Lemma 26.2 will
give i" ~ i'.
It remains to show that i' is a quasi-isomorphism. Let W = (i' — i")(V).
Then / = T(V ©W ® sV). Moreover the ideal I generated by W and sV is
preserved by d. Since / = TV ?? we need only show H(l) = 0. For this use
multiplication in / to write I = TV' <g> (W ® sV) ® /. Define h : X —>¦ X by
,x = i'v-i"v,
346 26 The dg Hopf algebra C.(QX)
Then a straightforward computation shows that hd + dh— id sends TV <g> W <8>I
into TV ®sV <g> / and sends TV' <g> sy <g> / to zero. It follows that #(I) = 0
and i' is a quasi-isomorphism.
Transitivity: Extend (I,d) to a chain algebra (J,d) as follows. Set J = T(V ©
V" © V" © sV © sV) where V" is a third copy of F and sV is a second copy of
the suspension of V. Regard i', i" and 5 as maps into J, let i'" : TV —> J be the
algebra inclusion extending the isomorphism V = V" and extend s : V -^ sV
to an (i",i'")-derivation 5 : TV —>¦ J. Define d in J by requiring ?"'" = i'"d
and dsu = ?"? - i'"v - ISdv, ? G I7·
Now suppose </>0 ~ ?? ¦ (TV,d) —> (A,d) and ?? ~ ?-2 : (TV,d) —> (A,d)
are dga homotopic by homotopies ? and ?'. Define a chain algebra morphism
? : (J,d) —> U»rf) by ?'?' = <^0, ?'*" = </>i, ?*'" = <^2, IIsu = ?? and
??? = ?'?. Define a chain algebra morphism ? : (J, d) —> (TV,d) by ??' =
7??" = ??'" = id and 7rsu = ttsu = 0, ? ? V. As in the proof of symmetry, ?
is a quasi-isomorphism and so Lemma 26.2 implies that i' ~ i"'. It follows that
</50 = ??' ~ II»'" = <y52- ?
(b) The dg Hopf algebra C*(Q.X) and the statement of the theorem.
Let (X, xq) be a based topological space, and recall (§2(b)) that UX is the
topological monoid of Moore loops at xo· Multiplication UX ? UX —> UX
is given by composition of loops and the identity is the constant loop c at xq.
Now suppose G is any topological monoid with multiplication, ?, and identity,
c. Then, as observed in the introduction to §16 for G = UX,
y"aig = ?,(?) ? ? ? : Ct(G) ® C,(C?) —> C,(G)
makes C»{G) into a chain algebra. On the other hand (§4(b)) the Alexander-
Whitney diagonal
Aalg = AW ? C.(Atop) : C,{G) -* C,(G) ® C.(C)
makes C,(G) into a differential graded coalgebra, where Atop denotes the topo-
logical diagonal sending y i-> (y. y).
Proposition 26.4 The maps /iaig and Aaig mate C*(G) into a differential
graded Hopf algebra with identity and augmentation given by
h = Ct({c}) —> C.(G) and C,(G) —> C,(pi) = A ·
proof: This is a straightforward calculation using properties D.4)-D.9). In
particular, the fact that Aaig is a morphism of chain algebras follows from the
compatibility D.9) of AW and EZ. ?
Lie Models 347
On the other hand, suppose (Ly,d) is a free Lie model for a simply con-
nected topological space X with rational homology of finite type (cf. §24). Then
U{Lv,d) is a differential graded Hopf algebra of the form (TV,d), whose co-
multiplication is the chain algebra morphism Av : (TV, d) —» (TV, d) <g> (TV, d)
defined by Ayv = ? ® 1 + 1 ® ?, ? G V* (§21(c), (f)). Our main theorem reads
Theorem 26.5 Let X be a simply connected topological space with rational
homology of finite type. The choice of a free Lie model (Ly, d) for X determines
a natural homotopy class of chain algebra quasi-isomorphisms
e-.U(Lv,d) -^C.(QX)
such that (? <8> ?)?? and Aaig ? ? are dga homotopic. Moreover ?(?) is the
isomorphism B6.1).
Remark Suppose (Ly, d) is a cellular Lie model (§24(c)) for a simply connected
CW complex X with rational homology of finite type. The quasi-isomorphism
of Theorem 26.5 has the form
(TV,d) 4?(??) ,
because t/Ly = TV (§21(c)). Moreover V is free on a basis corresponding to
the cells of A'.
This is therefore a special case of the classical Adams-Hilton model [2]. This
model, and the de Rham algebra of differential forms on a manifold, were the
first uses of differential graded algebras as a tool in homotopy theory. ?
(c) The chain algebra quasi-isomorphism ? : (ULv,d) -=> C,(FLy ,SL).
Fix an arbitrary free connected chain Lie algebra (Ly, d) of finite type. In
§25(a) we constructed a topological group |rLy,5jr| as follows. First, we let
(TLv,Sl) be the commutative graded Hopf algebra dual to [/Ly. Thus the
multiplication ??· and the comultiplication Ar are respectively dual to the co-
multiplication Au and the multiplication ?? in [/Ly. Then we applied the
spatial realization functor of §17(c) to obtain |FLy,i| = |(FLy,?i,)| with multi-
plication |??| and topological diagonal \??·\ (cf. Proposition 25.1). Since (Ly,d)
is fixed in this discussion we shall abbreviate the dg Hopf algebra (FLy,^z,) to
? so that our topological group is simply denoted by |?|.
As observed in Proposition 26.4, C, |?| is a dg Hopf algebra with multiplication
C»(|Ar|) oEZ and comultiplication AW ? C„(|/zrl)· However, included in C,\T\
is the sub dgc C»(T), as defined in §17(a). Since ? ? and AW are defined in
the wider context of simplicial sets (§17(f)), C*(T) is a sub dg Hopf algebra
of C,|r|, with multiplication /iaig = C(Ar) ° EZ and comultiplication Aa)g =
Now recall from §17(f) the natural morphism of chain complexes /e : C*{AV, d)
—> C(\v,d), dual to the integration operator § defined in §10(e). In the case of
(FLy, 5l) it takes the form
348
since ? is the dual of ULv ¦
26 The dg Hopf algebra ?.(??')
Proposition 26.6 The map Jt : C*(T) —» {ULv ,d) is a chain algebra quasi-
isomorphism.
proof: Proposition 17.16 asserts that J9 is a quasi-isomorphism. Proposi-
tion 17.17 asserts that
C, (?) ® C, (?) /-®/-v ULV ® ULv
commutes. But the multiplication |??| in |?| is the realization of
the dual of the multiplication ?? in ULv. Thus for ?,? e (?),
/.? - r = JmC*(&r)EZ(a ® r) = ??^??(? ® r) = /. ? · /, r .
which is
Recall now (§21(c)) that the universal enveloping algebra (C/Lv,d) is, as a
chain algebra, the tensor algebra (TV, d). Thus we may apply Lemma 26.2 to
the chain algebra quasi-isomorphism Jr : C»(r) —> (TV,d) to obtain a chain
algebra quasi-isomorphism
uniquely determined up to dga homotopy by the requirement that ft ? ? ~ id.
Lemma 26.7 The quasi-isomorphism ? commutes with the comultiplications
in ULv and in C»(F) up to dga homotopy.
proof: We have to show that
(? ® ?)?? ~ AW ? ?(?1??) ? ? .
Now here ?1?? is just (??), where ?? is the dual of ?[/. Consider the (non-
commutative) diagram
Lie Models 349
are dga morphisms, as follows easily from property D.7) and D.8) for EZ
and from property D.9) for AW. Moreover (Proposition 4.10) these are quasi-
isomorphisms, and AW ? EZ — id. In particular,
AW ? C, (??) ? ? = Aaig ?? = AW ? EZ ? Aaig ? ? .
Since AW is a chain algebra quasi-isomorphism we may apply Lemma 26.2(ii)
to conclude that C»(/ir) ° ? ~ ? ? ? Aaig ? ?.
Finally, note that the right hand square in the diagram above commutes by
naturality. Thus
Au ~ Au ? Jt ? ? ~ /? ? <7,(??) ? ? ~ /? ? ?? ? Aaig ? ? .
The diagram in the proof of Proposition 26.6 allows us to replace J oEZ by
Au ~ /, <8> /, ? Aaig ? ? .
Thus, since ft ? ~ id,
L ® /. ° o ® o ° At/ ~ At/ ~ /? ® /? ? Aaig ? (9 .
But /t is a quasi-isomorphism. Apply Lemma 26.2 to obtain
1 ? Au ~ Aaig OO. D
(d) The proof of Theorem 26.5.
We are given a free Lie model (Ly, d) for a simply connected topological space
X, which means that we have specified a cochain algebra quasi-isomorphism
m:C*(Lv,d) ^ APL{X) .
For simplicity of notation we shall denote (Lv,d) simply by L.
Suppose first X is a CW complex. Recall that ? = (FL, ?) denotes the dg
Hopf algebra Hom(UL,]k). Apply C, to the chain B5.3) of rational monoid
equivalences to obtain the chain
?^ B6.8)
of dg Hopf algebra quasi-isomorphisms.
Next, recall that UL = TV, and use Lemma 26.2 to lift the quasi-isomorphism
of §26(c) through this chain to obtain a chain algebra quasi-isomorphism
?: (UL,d) A
350 26 The dg Hopf algebra C.(iLY)
It commutes up to dga homotopy with the comultiplications because ? does
(Lemma 26.7) and because the quasi-isomorphisms in B6.8) commute with the
comultiplications. Moreover ? is uniquely determined up to dga homotopy and
hence, so is ? (Lemma 26.2). Since J is natural and the homotopy class of h ?
is natural in X (Theorem 17.15) so is the homotopy class of ?.
Now we have to show that ?(?) coincides with the isomorphism B6.1). We
may clearly suppose X = \C*L\, h ? = id and
m:C*L^> APL\C*L\
is one of the canonical homotopy class of morphisms (specified in §17(d)). Since
H{UL) = UH{L) it is enough to show these isomorphisms agree on [v] for any
cycle ? e L. Thus by naturality we are reduced to the case L = (L(u),0) is the
free Lie algebra on a single generator of degree n.
In this case the isomorphism B6.1) sends ? to the unique homology class
corresponding to [sv] under the isomorphisms
Hn (Q\C*L\) #- Hn+1 (P\C*L\, n\C*L\) ? Hn+l {\C*L\) .
But H(Q) has the same effect (use the fibrations B5.4) and a simple calculation
in C*{L;TL)). D
Exercise
Determine a chain algebra quasi-isomorphism ? : (TV, d) —> C» (fLY) when X
is a wedge of spheres 5"', n* > 2. Deduce from this the Hopf algebra structure
of Ht(QX;Q).
Part V
Rational Lusternik Schnirelmann
Category
27 Lusternik-Schnirelmann category
In this section the ground ring is an arbitrary commutative ring k, unless oth-
erwise specified.
A subspace ? of a topological space X is contractible in X if the inclusion
i : ? —y X is homotopic to a constant map ? —> xq.
Definition The LS category of X, denoted cat A", is the least integer m (or
oo) such that A' is the union of m + 1 open subsets ?/z, each contractible in X.
Remark LS category was originally introduced in [116], where it was shown
that cat X + 1 is a lower bound for the number of critical points of any smooth
function on a closed manifold X. The original definition differs from the one
above by 1; however the definition above has become standard in homotopy
theory because, clearly, cat A = 0 if and only if A" is contractible. Note that it
follows that
catS" = 1, ? >0 .
Moreover if xa G Xa has a neighbourhood contractible to xa in Xa then
cat ( V Xa ) = max {cat Xa }
\a J a
as is immediate from the definition.
This section is devoted to some of the basic geometric properties of LS cat-
egory. (For other presentations the reader is referred to [96], [62] and [40].)
For example, in §27(a) we shall prove that cat A' < d/r for an d-dimensional
(r — l)-connected CW complex, and in §27(f) we shall see that if cohomol-
ogy classes a; e H+{X;k) satisfy ?? U · · · U an ? 0, then cat A > n. Now
CPn is a 1-connected CW complex of dimension 2n whose rational cohomol-
ogy algebra has the form k[a)/an+l where ? G H2(CPn;Q). It follows that
? < catCP" < 2n/2; i.e.
catCP" =n .
An identical argument shows that the LS category of any simply connected
symplectic manifold A" (Example 5, §12(e)) is half the dimension.
However, our focus in this section is on a number of useful characterisations
of the inequality cat A" < m, including:
• deformation of the diagonal of Am+1 into the fat wedge (Whitehead).
• existence of a cross-section of the mth Ganea fibration (Ganea).
• retraction of A" from an m-cone (Ganea).
• A V ?? is an m-cone (Cornea).
352 27 Lusternik-Schnirelmann category
LS category is an invariant of homotopy type, but it is particularly difficult to
compute. For this reason it is useful to approximate it by other invariants. Thus
we introduce the cup-length, c(X;R) and Toomer's invariant, e(X;k), which
are more algebraic in nature, as well as the geometric cone-length cl X which is
the least m (or oo) such that X has the homotopy type of an m-cone. We shall
show that
c(A';A) <e(X;k) <catA <clA .
In this section we shall make heavy use of cones and suspensions. Thus we
recall (§ 1 (f)) that the cone on a topological space A' is the space CX = A" x
I/X x {0}, and that the points (x, t) are usually denoted by tx, 0 < t < 1,
with Ox the cone point. This identifies I = Ix {1} asa subspace of CX. If
g : A —>· X is any continuous map the adjunction space A Us CA is called the
cofibre of g.
Next define the reduced cone on a based space (A, xo) to be the space CX =
CX/Ixq. Then the suspension of X is the based space ? A = CA/A. If (A, x0)
is well-based then (CA, Ix0) is an NDR pair, the quotient map CX —> ~CX
is a homotopy equivalence (Corollary to Theorem 1.13) and (CA,.t0) and ??
are well-based. If, in addition, A is path connected a simple van Kampen argu-
ment shows that ?? is simply connected. Furthermore the long exact homology
sequence associated with the pair (CA, A) gives rise to natural isomorphisms
?{(??; Ik) A Hi-i(X; Jfe) , i > 2 .
We shall use all these facts freely without further reference.
This section is organized into the following topics:
(a) LS category of spaces and maps.
(b) Ganea's fibre-cofibre construction.
(c) Ganea spaces and LS category.
(d) Cone-length and LS category: Ganea's theorem.
(e) Cone-length and LS category: Cornea's theorem.
(f) Cup-length, c(A; Jfc) and Toomer's invariant, e(A; k).
(a) LS category of spaces and maps.
The definition of LS category extends from spaces to continuous maps as
follows:
Definition The LS category of a continuous map f : X —> Y, denoted cat/,
is the least integer m (or oo) such that A is the union of m + 1 open sets Ui for
which the restriction of / to each [7; is homotopic to a constant map Ui —> j/j.
Rational LS-Category 353
Note that
cat ? = cat id ?
so that this is a generalisation of the LS category of a space.
We say a topological space X is a homotopy retract of a topological space Y
if there are continuous maps X —> Y -^ X such that gf ~ id ?.
Lemma 27.1 Suppose f : X —> Y is a continuous map.
(i) If f ~ f then cat/' = cat/.
(ii) If g :Y —>.Z is continuous then cat gf < min(catc?, cat/).
(iii) If g (resp. f) is a homotopy equivalence then cat g f = cat/ (resp. cat gf =
cat^j.
fiw,) cat / < min (cat X, cat Y).
(v) If X is a homotopy retract of Y then cat A" < catY.
proof: (i) and (ii) are trivial consequences of the definitions. For (iii) suppose
g' is a homotopy inverse for g. Then g' gf ~ / and so cat/ = c&tg'gf < cat gf <
cat/. Similarly if/' is a homotopy inverse for / then cat g = cat gf f < cat gf <
cat g. (iv) follows from (iii) applied to idy of and / ? id ? and (v) is immediate
from (i), (ii) and (iv). D
As an immediate consequence of Lemma 27.1 (v) we deduce
Proposition 27.2 If X and Y have the same homotopy type then cat A' =
cat ?. ?
Lemma 27.3 For any continuous map g : A —> X,
cat(A Ug CA) < cat X + 1 .
proof: Let cat AT = m and put ? = [? ? {0}] e CA. Then A' Ug CA - {a}
is an open subset of A Ug CA containing A as a deformation retract. Hence
TO
cat (X Ug CA — {a}) = m and A Ug CA — {?} = (J Ui with Ui open and con-
o
tractible in A Ug CA. Since CA — A is open and contractible in A" Ug CA it
follows that cat(A Ug CA) < m + 1. ?
Next consider a based topological space (A, x) and denote its (m + l)-fold
product by Am+1 = Ax- · -x A. The/?? wedge, Tm+lX C Am+1 is the subspace
given by Tm+1(A) = {(x0,.. .,xm) e A"m+1 | some x{ = x). The diagonal, Ax :
X —> Xm+l, is the continuous map ?? : ? ?—)¦ (?, ?,..., ?).
Definition The Whitehead category of X, denoted Wh cat A", is the least in-
teger m (or oo) such that ?? is homotopic to a map / : X —> Tm+1(X).
354 27 Lusternik-Schnirelmann category
Proposition 27.4 [158] Suppose (X, x) is path connected
(i) If X is normal then Wheat.Y < catX.
(ii) If ? is contained in a subspace U that is open and contractible in X then
cat ?' < Wh cat X.
proof: (i) Let m = cat X so that X = (J Ui with Ui open and contractible in ?".
?
Because X is normal there are subspaces At C Oi C B{ C Ui with Ai, Bi closed
and Oi open and X = \JAi, and there are continuous functions hi : X —> I
such that hi I . ? 1 and hi \v „ ? 0.
Let Hi : Ui y. I —> X be a homotopy from the inclusion of Ui to a constant
map. Because X is path connected we may suppose Hi(—, 1) : Ui —> x. Define
Ki-.X x I—>X by
j ? , ? ? X -
Ki(x,t) = j
Then I<i(-, 1) : >li —> ? and so
? : ? ? / —> .Ym+1 , Uf(z, i) = (UfoU i)>..., Km(x, t))
is a homotopy from ? to a map X —» Tm+1(X).
(ii) Let m = Whcat(.Y) and let K(x,t) = (K0(x, t),... ,Km(x,t)) be
a homotopy from ? to a map / = (/0,.-.,/m) : A' —> Tm+1(X). Set [7, =
f^l{U). Then X = (J^i· Moreover ?"; is a homotopy from the inclusion of
?
Ui to the map /; : Ui —> U. Since U is contractible in X it follows that Ui is
contractible in X too. D
Corollary //A' is a path connected CW complex then
cat A" = Wh cat X .
proof: By Proposition 1.1 (iv) and (?), ? satisfies the two additional hypothe-
ses of Proposition 27.4. G
Recall next that a topological space X is c-connected if Ki(X) = 0, 0 < i < q.
Proposition 27.5 Suppose X is an (r — \)-connected CW complex of dimen-
sion d (some r > I). Then
cat.Y < d/r .
Rational LS-Category 355
proof: It follows from the construction of Theorem 1,4 that there is a weak
homotopy equivalence g : Y -=» X where Y is a CW complex whose (r — 1)-
skeleton is a single 0-cell, yo· By Corollary 1.7 this is a homotopy equivalence,
and hence has a homotopy inverse / : A" -^ Y.
Let m be the integer part of d/r, and recall from Example 3, §l(a) that ym+1
is a CW complex whose fc-cells are the products ?>?° ? · · · ? D^ of cells in
Y with ?&? = k. Since d < (m + l)r, the (i-skeleton of Ym+1 Ts contained
in Tm+1(Y). In particular, a cellular approximation (Theorem 1.2) of the map
?/ = (/,...,/): A' —» Ym+1 is a map h : X —» rm+1(F) such that ?/ ~ h.
Finally, since gf ~ id, g ? · · · ? g ? ?/ ~ ??, ?? the diagonal of A'. Thus
?? ~ (g ? ¦ ¦ ¦ ? g)h : ? —> Tm+1(X). By the Corollary to Proposition 27.4,
catX <m. D
(b) Ganea's fibre-cofibre construction.
Suppose (A", xo) is a based space and let j : F —> ? be the inclusion of the
fibre at xq of a fibration
p:E—>X.
Extend ? to the continuous map (cf. §l(f))
PC : ? Uj CF —> A"
by setting pc(CF) = x0.
Then (§2(c)) convert pc to the fibration
p : ?/ —> A
defined by ??' = (E Uj CF) ? ? Ai A and ?'B,7) = -?(?), ? the length of the path
7. According to Proposition 2.5, a homotopy equivalence A : ? Uj CF ^=> 2?'
is given by Az = (z,cPc2), cx denoting the path of length zero at x. Note
that p'X = pc- Precomposing A with the inclusion of ? gives the commutative
diagram
which is called Ganea's fibre-cofibre construction. It is obviously functorial with
respect to maps of fibrations.
Now consider the special case that ? : ? —> X is itself obtained by converting
a continuous map / : ? —> X into a fibration, so that
E = Z xx MX and F = ? xx PX .
356 27 Lusternik-Schnirelmann category
Thus a holonomy action is defined on F as well as on the fibre F' of p'. Recall
further from §l(f) that the join F * QX is defined by
F * ?? = (Fx CQX) UFxnX (CF ? ??) .
Right multiplication in ?? and the holonomy action in F define a diagonal
action of QX on F * ??((??,?) · g = (ug,vg)). More generally an ??"-space is
a topological space equipped with a right QX action and a map of ??-spaces
is a continuous map that commutes with the actions.
Proposition 27.6 (Ganea [63])
(i) The fibre F' of p' at x0 has the homotopy type of F * QX.
(ii) If ? is obtained as above by converting a map f to a fibration then there is
a homotopy equivalence F' -^ F*QX which is also a map of QX-spaces.
proof: (i) By construction,
F' = (E Uj- CF) xx PX = (Exx PX) U,- {CF ? ??) ,
i denoting the inclusion of Fx QX in ? ? ? PX.
Now in the proof of Proposition 2,5 (with different notation) we constructed
a continuous map ? : ? ?x PX ? [0, ??) —> ? such that ??(?, ¦y, t) = j(t) and
?(?,7,0) = ?. For 7 € QX let 7 be the loop of length ?7 given by 7(?) = ?{??—t).
Define ? : F ? ?? ? I —> ? ?? ? ? by setting
where 7 has length ?? and ?[ is the path of length ?? given by j't(s) =
7 (A - ?)?? + s). Then ? factors to give a map ? : F ? CQX —» ? xx PX.
Moreover, by construction K(y,j, 1) = (y',7) where y' = $(y, 7, ?7) e F. Thus
it extends to the map
(K, /) : (F ? CQX) UFxnx (CF ? QX) —» (? x* PX) U; (CF ? ??) = F' ,
where f(ty,j) = (ty',j) is obviously a homotopy equivalence.
But formula A.15) identifies (F ? 6??) UF*ax (CF x_QX) as F * ??.
Moreover, if cXo denotes the loop of length 0 at ar0 then ? restricts to the
inclusion F x {cXo} ? {1} —> ? ? ? PX, and this inclusion is a homotopy
equivalence by Proposition 2.5. Thus ? is a homotopy equivalence and hence
Theorem 1.13 asserts that so is (K,f).
(ii) As above. F' = (? ? ? ?X) U,· {CF x ??), with ?? acting by
multiplication on the right. Observe that composition of paths defines a homo-
topy equivalence MX ? ? ? ? -=» PX. This extends to a homotopy equivalence
Rational LS-Category 357
? xx PX = ? ?? MX ?? PX -=> ? ?? PX = F and hence (Theorem 1.13)
to a homotopy equivalence
?-.F'^FUa (CF ? ??) ,
where a : F ? ?? —> F is the action of ??.
If 7 G ?? has length ? define 7' e ?? to be the loop of the same length
satisfying ¦y'(t) = ?{? — t). Then 7 1—> 7' is a homeomorphism and the maps
7 1—> 7*7', 7 1—> 7' ·7 are homotopic to the constant map. It follows that a
homotopy equivalence m : CFxuX —> CFxuX is defined by (y, 7) 1—> A/7,7).
Denote by q0 : F ? ?? —> F and q\ : F ? ?? —> ?? the projections. Then
qi ·?? = a and so (Theorem 1.13)
id Urn : F Ua {CF ? ??) ^ F Ugo {CF ? ??)
is a homotopy equivalence.
By inspection the homotopy equivalence {id Urn) ? ? : F' -?» FUgo {CF x ??)
converts the action of ?? in F' to the diagonal action in CF ? ??. Since
F Ugo {CF ? X) = F Ugo (/ ? F ? ??) UCl UX = F * ?? ,
the proof is complete. D
(c) Ganea spaces and LS category.
Fix a based path connected topological space, {X, x0). Start with the path
space fibration, and iterate the fibre-cofibre construction to produce a sequence
of fibrations
PX
PiX
PmX
The space PmX is called the mth Ganea space for X and pm is called the mth
Ganea fibration. We set P0X = PX, Po = ? and we denote the inclusion of the
fibre of pn at x0 by jm : Fm —» .PmAr. Thus Fo = ?? and (Proposition 27.6)
Note also that if / : {Y, yo) —> {X, xo) is a continuous map of based path con-
nected spaces then the functoriality of the Ganea construction gives commutative
diagrams
PmY
Pmf
PmX
Y
- X
, m > 0 ,
358 27 Lusternik-Schnirelmann category
in which Pof = Pf.
Notice that
cat PmX < m . B7.7)
Indeed, since PmX — Pm-iX Ujm_j CFm_i we have
= cat (Pm-iX Ujm_, CFm_i) < catP^A" + 1 ,
by Proposition 27.2 and Lemma 27.3 respectively. Since P0X = PX is con-
tractible, B7.7) follows by induction.
Proposition 27.8 (Ganea [62]) The following conditions are equivalent on a
continuous map f :Y —> X from a normal space Y :
(i) f = pma for some continuous ? : Y —> PmX¦
(ii) f ~ pma for some continuous ? : Y —> PmX-
(Hi) cat f <m.
proof: (i) <i=?> (ii): Suppose q : ? —> X is any fibration and h,? '· W —» E,
hx : W —> X are arbitrary continuous maps such that qhs ~ ??. Lift the
homotopy starting at Jie to obtain Jie ~ h with qh = hx. In particular (i) <=$>
(ii).
(H) => (Hi): If (ii) holds apply Lemma 27.1 to conclude cat/ =
catpma < catpm < catPmX. Since catPmX < m by B7.7) it follows that
cat/ < m.
(Hi) => (ii): Denote the constant map by cy : Y —» {^o}. If cat/ = 0
then / ~ cy, which certainly factors through po- Suppose cat/ < m, some
m > 1. Then Y = \J Ui, where the Ui are open and f\u_ ~ cy\v , Since Y is
i=0 ' '
normal there are open subspaces Vo,V\ CY such that Y = VbU Vi and such that
m-l
the closures A and ? of Vo and Vi satisfy A C U Ui and ? C Um. Denote /I
and cy\A by /a and ca- Clearly cat/? < m — 1. Since A is closed it is normal
and so we may suppose by induction (because (i) <i=?> (ii)) that Ja = Pm-??? for
some ? a '· A —> Pm-\X, On the other hand, since ? C Um there is a homotopy
HB : ? ? / -^ X from f\B to cY\B.
Now construct a continuous map
?:??{0}?(??)???)???{1}^ Pm-\X
??{0}
Rational LS-Category 359
as follows. First, set ?\??<0-, = ??. Next, recall (§l(f)) that the points in
CFm_i are denoted by tz, t e I, z e Fm-i· Let v0 be the cone point: vQ = Oz
for all ? e Fm_i. Lift Hb to a homotopy ? : AnB ? I —> Pm-\X from ? a \AnB
to a map -4??-> Fm^1. Define ?\4nBx/ by
? H(y,2t) , 0<i< I .
<?<
Finally, set </?(? ? {1}) = v0.
Since Y is normal there is a continuous function h : Y —> I such that h(Y —
\\) = 0 and h(Y — Vo) = 1. Then y <—> (y,h(y)) defines a continuous map
a:F->Ax{O}U(An?x/)UBx{l}. Set
? = ???:?—> Pm-iX U CFm_, ^-^ PmX .
It remains to show that pma ~ /. Note that pm(CFm-i) = xo· Thus pm(p
extends to the continuous map ? :? ? {0} U ? ? ? —> X given by ip(y, 0) = fy
and, for y G B,
f HB(y,2t) , 0<?<|
The obvious deformation of y ? {0} U ? ? I to ? ? {0} provides a homotopy
from ????? = "?a to /. D
Corollary // X is a normal topological space then cat X < m if and only if
there is a continuous map ? : X —» PmX such that pmv = idx· ?
(d) Cone-length and LS category: Ganea's theorem.
The iterated suspensions ???? of a based topological space X are defined
inductively by
?°? = X and Y.kX = ???^?) , k > 1 .
They appear in the
Definition An ?-cone is a based topological space (?,??) presented in the
form
{po} = PoCPi C---CPn = P
where
Pk+1 = Pk Uhk ??*?· , k > 0 ,
for a sequence of well-based spaces (Yk,yit) and continuous maps hk : {EkYk,yit) —
(Pk,xo)· The (Yk,yk) are the constituent spaces of the cone and the hk are the
attaching maps.
360 27 Lusternik-Schnirelmann category
Remark 1 A simple van Kampen argument shows that if (P,xo) is an n-
cone with path-connected constituent spaces then each Pk (including ? itself) is
simply connected.
Remark 2 Note that Pi = ???, and so the 1-cones are simply the suspensions.
Remark 3 An ?-cone is automatically well-based since each (??'?^,?'?^
is an NDR pair.
In §l(a) we introduced a homeomorphism ?5* = Sk+1. In the same way we
have CSk = Dk+l. Thus an ?-dimensional CW complex X with a single 0-cell,
no 1-cells and based attaching maps hk '¦ Vq-^o —* Xk 1S a special case of an
n-cone:
xk+l = xk uhk
Definition The cone-length, cLY, of a topological space X is the least ? (or
oo) such that X has the homotopy type of an n-cone.
In this topic we establish two main results.
Proposition 27.9 (Ganea [63]) If(X,xo) is well-based then each Ganea space
PnX has the homotopy type of an n-cone with constituent spaces Yk — (QX)Ak+1.
In particular, if X is simply connected so is each PmX,
Theorem 27.10 (Ganea [62]) If X is normal then
cat AT < m <==>· ? is a homotopy retract of an m-cone.
In particular,
cat.Y <clAr .
Remark A recent result of Dupont shows that, even rationally, this inequality
can be strict; he constructs a rational CW complex X with catA7" = 3 and
clA' = 4.
proof of Proposition 27.9: Recall that pn : PnX —> X denotes the nth
Ganea fibration (§27(c)) and that the fibre inclusion at xq is denoted by jn :
Fn —> PnX- Let cI0 denote the loop of length zero at xo·
Step 1: (?.?, cI0) is well-based.
Choose a continuous h : X —> I, an open set xq G U C X and a homotopy
rel xo, H : U x I —> X from the inclusion of U to the constant map U —> Xq,
such that h~l(G) = xq and /?-1([0. ?]) C U. Define a continuous function k :
Rational LS-Category 361
?? —> / by ?G) = ?7 + sup {?G*) | ? G [0, oo)}, ?7 denoting the length of 7.
Let V C ?? be the open set of loops of length < 1 that lie entirely in U. If
7 G V let 7t be the loop of length it given by
i^s),») , 0<i<§. , f*r , 0<f<
7 \ x \ < t < 1 . ' \ B - 2?)?7 , I < ? < 1 .
Then k,V and ? : (-y,t) 1—> {it,it) exhibit (??,??0) as well-based.
Step 2: The smash products (??)?* = ?? ? · · · ? ?? (k times) are well-based
and ?"(??)??+1 ~ Fn.
proof: If (.4, uq) and (?, 60) are well-based then (.4 ? ?,? ? {b0} U {a0} x B)
is an NDR pair (Proposition 1.9). Hence ,4 ? ? is well-based. It follows by
induction starting with Step 1 that (??)?? is well-based. On the other hand,
Fn ~ Fn^i *CIX, as follows from Proposition 27.6. Since Fo = QX and since for
well-based spaces A * ? ~ ?(? ? ?) ~ ? ? ?? we have (by induction) that
Fn ~ (??)*<?+1) = (??)*(??)*? ~ ??*?"-1 ((??)??) ~ ?? ((??)??+1) .
Step 3: ??? has the homotopy type of an ?-cone with constituent spaces Yk =
(??)?*+1.
Recall from §27(c) that P0X = PX ~ {pt} and that PnX ~ Pn-iX Ujn_^
CFn_lt Choose h : ?"-1(??)?? ^ Fn^ (Step 2) and (by induction) / :
Pn-iX -^ Z, where ? is an (n—l)-cone with constituent spaces Yk = (??'')??"+1.
Set g = fjn-ih. Then Theorem 1.13 asserts that the maps
are homotopy equivalences. Moreover, since ??-1(??)?? is well-based
?7?"-1(??)?? ^ ???-1(??)?? and so, finally ??-?? Ujn_, CFn^ ~ ? Uh
???-'(??)??, for some continuous h : ?"-1(??)?? —» ?. Since the homo-
topy type of an adjunction space only depends on the homotopy class of the
adjoining map (Lemma 1.12) we may take h to be a based map. ?
proof of Theorem 27.10: Reduce to the case of well-based spaces (X, xo) by
replacing X by X Uz [0,1] and setting x0 — 1. If X is a homotopy retract of an
m-cone ? then catX < cat ? (Lemma 27.1(v)) and cat ? < m (induction using
Lemma 27.3). Conversely, if cat X < m then X is a retract of PmX (Corollary to
Proposition 27.8) which has the homotopy type of an m-cone (Proposition 27.9).
The final assertion of the Theorem follows immediately from this equivalence.u
(e) Cone-length and LS category: Cornea's theorem.
The purpose of this topic is to establish Cornea's remarkable strengthening of
Theorem 27.10:
362 27 Lusternik-Schnirelmann category
Theorem 27.11 (Cornea [40]) If X is a normal topological space then
cat ?' < m 4=> X V ?,? has the homotopy type of an m-cone
for some m — 1 connected space Y.
Remark In fact, Cornea shows that there is an m-cone of the form X V Emlr'
and deduces that cl A' < cat X + 1. We will not prove this refinement here since
rationally it is immediate and since it requires a result from homotopy theory
not included in the text.
In proving Theorem 27.11 we shall need to identify spaces as having the ho-
motopy type of ?-cones. For this we shall reply heavily on the following.
Basic Facts:
• // (Y, A) is an NDR pair then the homotopy type of ? U/ Y depends only
on the homotopy class of f : A —> ? (Lemma 1.12).
• // (?',?1) is a second NDR pair and if f : A' —> Z' then compatible homo-
topy equivalences (?,?,?) —>· (?1, Y', A') induce a homotopy equivalence
? U.4 ? ^ ?' LU- Y' (Theorem 1.13).
• // (Y, A) is an NDR pair and A is contractible then the quotient map Y —>
Y/A is a homotopy equivalence (Corollary to Theorem 1.13).
Now suppose
q:E—>B
is a fibration with fibre inclusion j : F —> ? at some base point b0 G ?. The
key step in the proof of Theorem 27.11 is
Proposition 27.12 Suppose ? has the homotopy type of an ?-cone with con-
stituent spaces Y/.. Then ? U,· CF has the homotopy type of an ?-cone whose
kth constituent space has the homotopy type of (Yjt ? F) U CF.
proof:
Step 1: Reduction to the case that ? is an n-cone.
Let g : ? —> ? be ? homotopy equivalence from an ?-cone ? with constituent
spaces Yk· Then (Z, zq) is well-based. Since ? is path connected we may replace
g with a homotopic based map; i.e. we may assume g : (?,??) —> (?,bo).
In the pullback diagram
ZxBE ^ ?
?
Rational LS-Category
363
gs is a homotopy equivalence because g is (Proposition 2.3). By our second
'Basic Fact' above. (? ? ? ?) U,· CF -=> ? U,- CF; i.e. we may assume ? = ? is
an ?-cone with constituent spaces Y^.
Write ? = W U/ CA where (W, wo) is an (n - l)-cone with constituent spaces
Yk, k < n, (A,a0) = (?'??,??) and / : (A,a0) —» (??,??). Let g ; ?,,t.·· —> PF
be the restriction of the fibration q to W.
Step 2. There is a homotopy equivalence of the form
Ew U9 (CA xF) ^> ?
in which ? : A x F —> ?\? restricts to j in {ao} x F.
Let (?, /) : (CA, A) —> (B, W) be the canonical map, and use it to pull the
original fibration back to a pair of fibrations
(CA,A) xBE
(?,?? )
(CA, A)
(E,EW)
(B,W),
noting that (??, Ie) is projection on (E, E\y) and (?, ? a) is projection on (CA, A).
The fibre of ? at ao is just f — {ao} x j : {a0} x F —» CA ? ? ?. Since CA is
contractible, Proposition 2.3 asserts that f is a homotopy equivalence.
Now let pL : CA x F —» CA and pR : ? ? ? F —» F be the projections, and
consider the (non-commutative) diagram
CAxF
j'p"
CA-x.bE
We construct a new map h : CA x F —> CA ? ? ? such that ph — pL,
h\r }xF = j' and both h and its restriction hA : A x F —> ? ? ? ? are
homotopy equivalences. For this we recall (Proposition 2.1) that a fibration has
the lifting property with respect to any DR pair and in particular with respect
to a pair of the form (? ? ?, ? ? {0} Ul" x /) where (Y, Y') is an NDR pair. We
also recall (Proposition 2.3) that the pullback of a fibration over an NDR pair is
an NDR pair. _ _
Choose a based homotopy ? : CA ? I —> CA from the constant map CA —>
{ao} to id-cA. Lift ? ? (pL ? id/) to a homotopy rel {ao} x F starting at j'pR.
Define h to be the restriction of this homotopy to CA x F ? {1}. Then ph = pL
and so h restricts to h a. : A x F —> A X? F. Also h restricts to j' in {ao} x F
and so h is a homotopy equivalence.
364 27 Lusternik-Schnirelmann category
It remains to show hA is a homotopy equivalence. As above, modify an arbi-
trary homotopy inverse of h to obtain a homotopy inverse h' satisfying pLh' = p.
Let p' : CA x I —> CA be the projection. If If is a homotopy from hti to
idcAXBE, then there is a homotopy rel CAx?E ? {0,1} from pK to p'(p ? idj)
because CA is contractible. Lift this to obtain ? ~ K' rel CA ? ? ? ? {0,1}.
Then ?' is a homotopy from hh' to "%???? and pK' = p'(pxidj). In particular
K' restricts to a homotopy hAh'A ~ idAXBB. Similarly, hAh,A ~ idAxF.
Now set ? = fEhA : Ax F —> Ew. Since (trivially) ? = Ew U/E (U.4 x??)
our second 'Basic Fact' gives
(id, h) : Ew ?? (CA xf)A Ew USb (CA xBE) = E .
Step 3: Completion of the proof of the proposition.
Recall the notation established at the end of Step 1. Since the map h of Step 2
restricts to f in {ao} x F it follows that ? = f?hA restricts to j : {ao} xF —y E.
Extend ? by idcF to a map g : Ax FU {ao} x CF —> Ew U,· CF. By our second
'Basic Fact' we may adjoin CF to both sides of the homotopy equivalence of
Step 2 and still have a homotopy equivalence. This may be written in the form
(Ew Uj CF) Ug (CA x F U {aQ} ? CF) ^ ? us CF .
By induction Ew Uy CF has the homotopy type of an (n — l)-cone, (D,do)
with constituent spaces of the homotopy type of Yk x F U CF, k < ? — 1. Use
the second 'Basic Fact' to obtain
D Ug' (CA x F U {ao} ? CF) ~ ? Uj CF ,
for a suitable map g' : ? ? FU {a0} ? CF —> D. Choose a well-based space F'
of the same homotopy type of F (e.g. F' = FUy [0,1]). Then
CAxFU {a0} xCF~CAxF'u {a0} x CF' ~CAx F'/{aQ} x F' .
Thus our 'Basic Facts' give a homotopy equivalence of the form
D Ufc (CA ? F'/{a0} x F') ~ ? Uj CF ,
where k : A x F'/{a0} x F' —> D is a based map.
Finally, recall (§l(f)) that the points of CA are denoted by ??, ? € ?, ? € I.
Define a homeomorphism CA x F'/{a0} x F' A C (A x F'/{a0} x F') by
(ta,y) ?—> t(a,y). Dividing by A x F'/{ao} x F' on the left yields a home-
omorphism ?? ? F'/{a0} ? F' ^?(?? F'/{a0} x F'). Thus
D Uk (CA ? F'/{a0} xF') = D UkC (A x F'/{a0} x F1)
and
A x F'/{a0} xF' = ?"-1^..! ? F'/{a0} x F' S ?"" (Yn^ x F'/{a0} x F1) .
Rational LS-Category 365
Since ??-? ? F'/{a0} xF' ~Yn-XxF'U CF' ~ Yn^ xFuCF, the inductive
step is complete. D
proof of Theorem 27.11: If A" V ?1' has the homotopy type of an m-cone
then X is a homotopy retract of an m-cone and cat X < m (Theorem 27.10).
Conversely, suppose cat ?" < m. Then there is a continuous map ? : X —>
PmX such that pma = id ? (Corollary to Proposition 27.8). Convert ? to the
fibration q : X Xpmx M(PmX) —> PmX as described in §2(c). Denote X xpmx
M(PmX) by ? and denote the inclusion of the fibre of q by j : F —> ?. Since
PmX has the homotopy type of an m-cone (Proposition 27.9) it follows that
? Uj CF has the homotopy type of an m-cone too (Proposition 27.12). On the
other hand, as observed in §2(c) there is a homotopy equivalence A : A" -^» ?
such that q\ = ?. Let A' be a homotopy inverse for A. Then \pmq ~ ApmaA' =
AA' ~ id?. Hence j ~ \pmqj, which is a constant map. By our first basic fact
"EUj CF ~ EVCF/F. But CF/F ~ ?? for any well pointed space Y homotopy
equivalent to F. Thus
A' V ?? ~ ? V ?? ~ ? Uj CF ,
and ? Uj CF has the homotopy type of an m-cone.
Finally, it is relatively easy to show that F ~ flFm. However, here we simply
notice that since pma = id ? it follows that 7r,(PmA') = ?* (A") (Bn*(Fm). More-
over 7r,(g) is an isomorphism of ?*(?) onto the subgroup identified with ??,(?').
It follows that the connecting homomorphism for the fibration q maps K*(Fm)
isomorphically onto tt^^F). Since Fm ~ Em(OAT)Am+1 and ??' is path con-
nected we may conclude that Fm is simply connected and that Hi{Fm) = 0,
1 < i < m. By the Hurewicz theorem 4.19, Fm is m-connected. Hence F and Y
are m — 1 connected. ?
Again, consider a fibration
q: ? —> ?
with fibre inclusion j : F —>· ? at some base point bo G B. From Proposi-
tion 27.12 we deduce
Proposition 27.13 If ? is normal then
d(E Uj CF) < cl ? and cat(? U,· CF) < cat ? .
proof: The first assertion is immediate from Proposition 27.12. For the second,
let cat? = m. Then ? is a homotopy retract of an m-cone ? (Theorem 27.10).
If ? -U ? -^? ? satisfy rf ~ idB then the homotopy lifts to a
366 27 Lusternik-Schnirelmann category
h ~ ids such that qh = rf. Thus we have maps of fibrations
?
?
(fg,fi).
f
?
which exhibit ? U CF as a homotopy retract of (? ? ? ?) U CF. Since P is an
m-cone so is (P xB E) U CF, by Proposition 27.12, and so cat? U CF < m. D
Example 1 ITie LS category of the Ganea spaces PnX.
Suppose X is a normal topological space with catX = m, m < oo. We show
that
in if ? < m
m if ? > m.
In fact, since Pn+xX ~ PnX U CFn it follows that catPn+iX < catPnX +
1 (Lemma 27.3). On the other hand, X is a retract of PmX (Corollary to
Proposition 27.8) and thus catPmA" > catX = m. Since P0X is contractible
catPo^ = 0 and now the first inequality implies catPnX = ?, ? <m.
On the other hand, Proposition 27.13 gives ca.tPnX < catA", all n. Thus
since X is a retract of PnX, ? > m we have catX < catPnX < catA", ? > m.D
(f) Cup-length, c(X; k) and Toomer's invariant, e(X;jk).
The cup-length, c(X; Jk), of a path connected topological space X is the great-
est integer ? such that there are cohomology classes a\,...,an G ?+{?;$?)
such that ?? U · · · U an ? 0. Toomer's invariant, e(X; Ik) is the least integer ?
for which there is a continuous map / : ? —> X from an ?-cone ? such that
H*(f; ]k) is injective. (This invariant was introduced in a slightly different form
by Toomer in [149].)
Proposition 27.14 If X is a path connected normal space then for any coef-
ficient ring fc,
c{X;k) < e(X-]k) < catX < cLY .
proof: To show c(X;Jk) < e(X;Jk) it is enough to show that c(Z\lk) < ?
for ?-cones Z. Write ? = ? Uh CA for some (n — 1) cone Y, choose classes
a0,. -., an € H+(Z; ]k) and let ? = ???· · Uan_i- Recall (§5) that the inclusion
C*(Z,Y;k) —> C*(Z;lk) induces a morphism A : H*(Z,Y;Jk) —> H*(Z;Jk) of
H*(Z;Jk)-modules. Now by induction ? restricts to zero in H*(Y;k) and so
? = ??', some ?' € ?* (?, Y; M). Hence ? U an = ?(?' U an).
Rational LS-Category 367
On the other hand, by excision the obvious map (?, h) : (CA, A) —» (?, ?)
induces an isomorphism ?*(?,?) : H*(Z,Y;Jk) -=» H*(CA, A;Jk). Moreover
?*(?,?)? U an) = H*(<p,h)?' U ?*(?)?? = 0, because CA is contractible.
Thus 0' U an = 0 and hence so is 0 U an = \{0' U an). This proves that
c(Z;M) <n.
The remaining inequalities are trivial consequences of Theorem 27.10 (where,
indeed, the third inequality is stated). D
Next recall the Ganea fibrations pn : PnX —> X introduced in §27(c).
Proposition 27.15 If (X.x0) is well-based and normal then e(X;Jk) is the
least integer m (or oo) such that H*(pm;Jk) is injective.
proof: Clearly e(X; Jk) is less than or equal to this least integer, because PnX
has the homotopy type of an ?-cone (Proposition 27.9). On the other hand, if
e(X;Jk) = m choose a map / : ? —> X from an m-cone such that H*(f;Jk) is
injective. We can suppose / is a based map because ? is well-based. (Replace
/ by a homotopic map if necessary.)
Recall (§27(c)) that / induces continuous maps Pmf : PmZ —> PmX such that
Pm °Pmf = }°Pm- Moreover, since ? is an m-cone cat ? < m (Theorem 27.10)
and so there is a continuous map ? : ? —> PmZ such that p^ ? ? = id ?- Then
/ = / °Pmocr = Pmo pmf ° ?. Since H*(f; k) is injective so is H* (p?; Jk). ?
Next, consider a continuous map
between topological spaces, and recall that it induces maps
PnY ^-^ PnX
I*
? 7 . ?
between the Ganea fibrations. If ? * (/; k) is an isomorphism then clearly c( Y ; .&) =
c(JY; h). However, we also have
Proposition 27.16 Suppose X and Y are normal and simply connected, and
let Jk cQ. If H*(f;Jk) is an isomorphism then e(Y;Jk) = e(X;k).
Remark There can be no analogue of Proposition 27.16 for LS category. In-
deed, the inclusion of the base point, xo, in the space X below is a weak homotopy
equivalence:
368 27 Lusternik-Schnirelmann category
X
However, no neighbourhood of x0 is contractible in X and so cat X = oo!
The main step in the proof of Proposition 27.16 is
Lemma 27.17 Suppose Jk C Q and H*(f;Jk) is an isomorphism. Then
H*(Pmf;Jk) is an isomorphism for m > 1. In particular if f is a weak ho-
motopy equivalence so is each Pmf.
proof: Suppose first that if /1.4 : A' —> A and hg '· ?1 —> ? are continuous
maps between path connected spaces, and that Ht(fiA',lk) and Ht(hB',lk) are
isomorphisms. Then the natural homeomorphism (CA ? ?) XaxB {A x CB) =
.4 * ? of §l(f) implies that H,(Iia * ??; $:) is an isomorphism.
Let Fmf : FmY —> FmX denote the restriction of Pmf to the fibres. Proposi-
tion 27.6 provides homotopy equivalences Fm(—) ~ Fm_i(—) *?(—) which, with
a little care, can be chosen to identify Fmf with Fm_i/ * Qf up to homotopy.
The Whitehead-Serre theorem 8.6 asserts that H*(uf;]k) is an isomorphism.
Hence so is each H*(Fmf; ]k).
A simple van Kampen argument shows that the join of path connected spaces is
simply connected. In particular, it follows from Theorem 8.6 that each Tr*(Fmf)®
Jk, m > 1, is an isomorphism. Hence PmX is simply connected, m > 1 and
7T«(-Pm/) ® Jfe is an isomorphism (long exact homotopy sequence). A second
application of Theorem 8.6 gives that Ht(Pmf;k) is an isomorphism. The final
assertion of the lemma follows immediately from the same theorem. D
proof of Proposition 27.16: If g : W —» ? is a continuous map and if
H*(g; k) is an isomorphism then C«(p; ]k) is a chain equivalence (i.e., has a chain
inverse) and so C*(g;lk) is a quasi-isomorphism too. In particular. H*(f;]k)
and H*(Pmf\Jk) are isomorphisms. Now use the construction X Ux [0,1] to
reduce to the case / is a based map between well-based spaces. Then apply
Proposition 27.15. D
Exercises
1. Let X and ? be based path connected spaces, and suppose catZ < m. Prove
that every continuous map f : ? —> X factors up to homotopy through Pm(X).
Rational LS-Category 369
2. Let X be a simply connected CW complex and Y = X U^ en. Suppose that
cat X = m and ? < 2m. Prove that csXY < csXX.
3. Prove that P^X) * ???.
4. A co-H-space is a space X together with a continuous map V : ?" -4 X V X
such that (idx V *) ? V ~ idx and (* V ???) ? V ~ idx. Prove that a based CW
complex X is a co-H-space if and only if catX < 1.
5. Let X be a finite CW complex. Prove that cat0X = cat,Yp, where ? is the
complement of a finite set in the set of all prime numbers (cf. §9-exercise 5).
28 Rational LS category and rational cone-length
In this section the ground ring is Q.
We begin by recalling some basic facts from §8 and §1 that will be used without
further reference; then we introduce cato, cl<j and e<j as (geometric) invariants
and finally outline the main results of the section, which treats rational category
from a geometric perspective.
Firstly, if / is a continuous map between simply connected topological spaces
then H, (/; Q) is an isomorphism if and only if ?» (/) <g> Q is an isomorphism
(Theorem 8.6). In this case / is a rational homotopy equivalence. Two spaces X
and Y have the same rational homotopy type if they are connected by a chain
of rational homotopy equivalences in alternating directions (Proposition 9.8), in
which case we write X ~q Y.
A simply connected space is rational if its homotopy groups (or, equivalently,
its integral homology groups) are rational vector spaces. For each simply con-
nected space X there is a relative CW complex {Xq, X), unique up to homotopy
type rel A' such that A'q is a rational space and A' —> Xq is a rational homo-
topy equivalence (§9(b)). We call such an Xq a rationalization of X. If X is a
CW complex then so is A'q. Finally if g : X —> Y is any continuous map into
a simply connected rational space Y then g extends (uniquely up to homotopy
rel X) to a map pQ : A'q —>· Y (Theorem 9.7).
Definition Let X be a simply connected topological space.
1 The rational LS category, cat0 X, is the least integer m such that Ar ~q Y
and cat y = m.
2 The rational cone-length, cl0 A, is the least integer ? such that X ~q Y
and cl(F) = n.
3 The rational Toomer invariant, eQX, is the least integer r such that X ~q
Y and e(Y; Q) = r.
4 The rational cup length, cqX, is the cup length of H*(X;Q).
Note that, by definition, these are invariants of rational homotopy type.
Among the main results of this section we establish
• For simply connected CW complexes X, cato A = cat A'q, cl0 X = cI(Aq)
and e0X = e(A;Q).
• For any simply connected space X,
e0X < cato Ar < cl0 A" < cat0 X + 1 .
• (Mapping Theorem) If a continuous map f : A' —> Y between simply
connected spaces satisfies: Ti*(f) ® Q is injective, then cato A < cato Y¦
This section is organized into the following topics:
(a) Rational LS category.
Rational LS-Category 371
(b) Rational cone-length.
(c) The mapping theorem.
(d) Gottlieb groups.
(a) Rational LS category.
Here we prove
Proposition 28.1 If X is a simply connected CW complex, then
(i) catoA' = cat.YQ.
(ii) e0X = e(X;Q) = e(XQ;Q).
First we need
Lemma 28.2 Let X be a simply connected CW complex.
(i) If f : X —» Y is a weak homotopy equivalence then cat X < caXY.
(ii) catA'Q < catX.
proof: (i) Put cat F = ??. Then cat/ < m (Lemma 27.1). Hence / factors
asp^off for some continuous ? : X —> PmY (Proposition 27.8). Since Pmf :
PmX —> PmY is also a weak homotopy equivalence (Lemma 27.17) we may lift
? through Pmf to construct a continuous ? : X —> PmX such that Pmf °? ~ ?.
Then / ? ?^ ? r ~ / and so pj? ? r ~ id ? (Lemma 1.4). It follows (Proposition
27.8) that catAr < m.
(ii) If cat ?' = m then there is a map ? : ?' —> PmX such that
?* ? ? = id (Proposition 27.8). This extends to aq : Xq —>· (PmX)q, and
(Pm)Q°*-Q~U/.
On the other hand, let j :'X —> Xq be the inclusion. A simple calculation
(using Fm ~ ???(??)???+? of Step 2 in Proposition 27.9) shows that Pm (Xq) is a
rational space. Hence Pm(j) extends to a continuous map Pm(j)Q ¦ Pm(X)Q —>
and Pm" °Pm(j)Q ~ {Pm)n· Since Xq is a CW complex it follows that
~ id and cat Xq < m (Proposition 27.8). D
proof of Proposition 28.1: Suppose X ~q Y. Choose a weak homotopy
equivalence ? —> Y from a CW complex Z. Then Xq and Zq have the same
weak homotopy type. But these are CW complexes and so they have the same
homotopy type. Hence cat^Q = cat^Q < cat ? < cat F.
On the other hand, Proposition 27.14 states that e(Y; Q) = e(Z; Q) and Propo-
sition 27.15 identifies this as the least integer m such that H*(p^l;Q) is injective.
Rationalizing PmZ and Z, we can replace p^ with (^)„. But it follows from
372 28 Rational LS category and rational cone-length
the proof of Lemma 28.2(ii) that if H* ( (p^) Q; <Q>) is injective so is H* (p^ ; Q) .
Thus e(XQ; Q) = e(ZQ; Q) < e(Z; Q) = e(Y; Q). D
Example 1 cat0 [\/ Xa) = max{cat0Xa}.
Vu / a
Here the Xa are taken simply connected. We may suppose the Xa are rational
CW complexes and then the equality follows from Proposition 28.1 and the
analogous equality for cat (introduction to §27). D
(b) Rational cone-length.
As a special case of the ?-cones denned in §27(d) we define spherical n-cones
to be based spaces (P.po) presented as {po} = Po C Pi C · · · C Pn with each
Pk+i — Pk Uhk CSk, where Sk has the form
Sk = V Sr°+k+1 , ra > 0 .
a€Jk
(In particular any composite of ? +1 Steenrod operations vanishes in a spherical
?-cone. Thus, although ECP°° is a 1-cone, it is not a spherical ?-cone for any
n.) Notice that each Pk in a spherical ?-cone is automatically simply connected.
Proposition 28.3
(i) If X is simply connected then clo X is the least integer ? such that there is
a rational homotopy equivalence f : ? —» X from a spherical ?-cone, P.
(ii) If X is a simply connected CW complex then clo X =
Lemma 28.4 If ? : X —>· Q is a rational homotopy equivalence to a simply
connected ?—cone, Q, then there is a rational homotopy equivalence f : ? —» ?'
from a spherical r-cone, P.
proof: Write Q = Qr D · · · D Qo = {q0} with Qk+i = Qk U5fc CT.kYk as in
§27(d).
Sublemma We may suppose each Qk is simply connected.
proof of sublemma: It is sufficient (van Kampen) that Yq be path connected.
Now Qi is simply connected because Q is, and so Hi(Q\;Z) = 0 (Theorem 4.19).
It follows that #?(??) : ?1(??1;?) —» ??(??0;?) is surjective, since this may
be identified with the connecting homomorphism iI2(<2i,Qo;Z) —> Hi(Qo\Z).
Next, recall that Yq and Y\ are well-based with base-points yo and y\. Let yoa
and yi? be base-points for the other path components of Yq and Y\. Then the
interval through yoa becomes a circle in ?^? representing a homology class ??,
and ?\(???',?) = 0???, again by van Kampen and Theorem 4.19. Similarly
Rational LS-Category 373
??(???;?) = @Zw0 where W? is represented by the interval through yl0. In
0
particular, we may choose a subset J of path components of Yl such that
Now for each yOa adjoin an interval Ia to Yo by attaching {0} to yo and {1}
to you· This gives Yo = V U ( ? Ia ). Similarly, construct \\ = ?? U ? ? ??\.
Va J \0eJ ¦ J
Then Yo is path connected, so ??0 is simply connected and pi extends to a
continuous map ?? : ??? —> ??0. Set Q = ??? Up, ???? U ??'2?2 U · · · U
???~???-\. Then Q is simply connected and a straight forward calculation
shows that the inclusion Q —> Q induces an isomorphism of rational homology.
Thus we may replace Q by Q; i.e., we may assume Yo is path connected, and
each Qk is simply connected. D
We now return to the proof of the lemma. Observe that it is sufficient to
consider the case that ? is a fibration. Set Xk = Q~1(Qk)- Since ?«(?) ®Q is an
isomorphism the fibre, F, of ? satisfies it» (F) <g> Q = 0. Hence the restrictions
Qk ¦ Xk —>· Qk satisfy ?*{?^ ® Q is an isomorphism.
Suppose by induction on k that there is a continuous map ? : ? —>· Xk with
? homotopy equivalent to a spherical k-cone, Pk and 7r»(p)®Q an isomorphism.
Again we may take ? to be a fibration. Theorem 24.5 provides a rational homo-
topy equivalence h : Sk —> ?*?* with Sk = V Sk+1+r". Let ha = h
Then the map C5t+1+r° -^- CEfcFfc —» Qk+i lifts through ?fc+1 to a based
map Ha ¦ C5fc+1+r» —» ??+?, and this restricts to a map OQ : 5A+1+r° —> XA.
Since 7r*(p)<g>Qis an isomorphism, there is a positive integer ma with mQ[oQ] G
Im7r»(p). Moreover, without loss of generality we may suppose ma = 1· (Simply
replace h by a new map h' so that [/iQ] = ma[ha]; then relabel /?' as h.) Then,
since ? is a fibration, ?? lifts to a based map ?? : Sk+1+ra —> Z, and we may
construct
? = (?, {Ha}) : ? U{(To} UV5fc+1+r» -* Xk+i .
a
Since ?, (?fcp) ® Q is an isomorphism so is H* ^kg; Q). Now an easy homology
calculation shows that the composite of ? with ?^\ is a rational homotopy
equivalence. Hence 7r„(p) ® Q is an isomorphism, and the lemma follows by
induction. ?
proof of Proposition 28.3: (i) Given a rational homotopy equivalence / :
? —> X from a spherical ?-cone we have clo(Ar) < cl(P) < n. Conversely,
suppose clo(X) = n. Then there is a chain of rational homotopy equivalences
? _> ...<_ Q
374 28 Rational LS category and rational cone-length
with Q an ?-cone. Apply Lemma 28.4 a finite number of times to obtain a
rational homotopy equivalence / : ? —> X with ? a spherical n-cone.
(ii) By definition cl0 A' < cI(A'q). Moreover, if Y ~q X and cl(F) = ? then
(Lemma 28.4) there is a rational homotopy equivalence ? —> Y from a spherical
?-cone. Present ? as {p0} C · - · C Pn = ? with Pfc+Z = Pk U CY.kYk, where Yk
is a wedge of spheres. Then there is an obvious weak homotopy equivalence
{po} U CT(n)Q U · · · U CE" (rn_i)Q -> Pq .
Denote the ?-cone on the left by P'. Since each {Pk+i,Pk) is a relative
CW complex and since Pq —> Xq is a weak homotopy equivalence a direct
application of Lemma 1.4 shows that P' —> Xq is a homotopy equivalence. Thus
cl(AQ) < ?. It follows that cl(.YQ) < cl(F) for all Y ~q X; i.e. cl(AQ) = cl0 X.
a
Theorem 28.5 If X is a simply connected topological space then
(i) e0X < cato X < cl0 ?' < cat0 A' + 1.
(ii) If e0X = 1 then e0X = cato X = clo X = 1 and X has the rational
homotopy type of a wedge of spheres.
(Hi) If X is a CW complex then cato AT < m if and only if Xq is a homotopy
retract of an m-cone.
proof: (i) Since the invariants are invariants of rational homotopy type we
may suppose A is a CW complex, so that eoAT = e(AQ;Q), cato A' = catXQ
and clo X = cI(Aq). (Proposition 28.1 and 28.3). Now the first two inequalities
of (i) follow from Proposition 27.14.
For the last inequality and the proof of (iii), let catoAr = m. We lose no
generality in supposing A a rational CW complex. Then A" ~ Xq and cat A" = m
(Proposition 28.1). Thus the mth Ganea fibration pm ¦ PmX —> X admits a
cross-section ? (Corollary to Proposition 27.8).
Convert ? to a fibration ? —> PmX with fibre F, and ? ~ A". In the proof
of Theorem 27.11 we showed that
? U CF ~ X V ?? ,
where Y is a well-pointed space homotopy equivalent to F. Hence A ~ (E U
CF) U ???.
On the other hand, we also showed in the proof of 27.11 that k*(F) =
7r*+i(Fm), Fm the fibre of pm. But Fm ~ Em (UXAm+1) by Step 2 in the
proof of Proposition 27.9. Since the smash of path connected well-based spaces
is simply connected (trivial) it follows that F and Y are m-connected. Finally,
Proposition 27.9 identifies P?nX as homotopy equivalent to an m-cone and so
EUCF also has this property (Proposition 27.13). Since X ~ (EUCF) ????,
it follows that cl0 A < cLY < m + 1.
Rational LS-Category 375
Note that we have identified A' as a retract of PmX which is homotopy equiv-
alent to an m-cone.
Finally to prove (ii) suppose e0X = 1. Then the first Ganea fibration,
??.? —>· X, is surjective in rational homology. Since there is a rational ho-
motopy equivalence from a wedge of spheres to ??.? (Theorem 24.5) the ratio-
nal Hurewicz homomorphism for X is surjective and X itself has the rational
homotopy type of a wedge of spheres (Theorem 24.5). D
Remark As observed in §27, Theorem 28.5(i) is a special case of a theorem of
Cornea.
(c) The mapping theorem.
The usefulness of rational LS category in rational homotopy theory is in large
part due to
Theorem 28.6 (Mapping theorem) Let f : X —)¦ Y be a continuous map
between simply connected topological spaces. If 7?, (/) <g> Q is injective then
cato X < cato Y ·
proof: We lose no generality in assuming X and Y are rational CW complexes.
Let cato Y = m and convert / to the fibration g : ? = ? ? ? MY —> ? as de-
scribed in §2(c). Then inclusion of the fibre ? ? ? PY induces zero in homotopy,
because nt(g) is injective. The homotopy equivalence ? —> X converts this
inclusion to a fibration ? : ? ? ? PY —> X and so ?, (?) = 0 and the fibre
inclusion j : QY —» ? ?? PY satisfies: n*(j) is surjective.
Decompose the rational vector spaces ?,,(??') as ?^ © ?'?, with tt,(j) : ?'? ^»
??(? xY PY). Let K'n and ?'? be cellular Eilenberg-MacLane spaces of types
{?'?,?) and (??^',?), respectively. Apply the Corollary to Proposition 16.7 to
obtain a weak homotopy equivalence
Then setting ?' = f\ K'n we see that ?? : ?' —> ? ? ? PY is a weak homotopy
?
equivalence.
Now let A : ? ? ? PY —>· ? be the inclusion and set
? = (id, Cjtp) : ? l)Xjtp CK' —> El)x C(X ? ? PY) .
By Proposition 27.13, cat (E U C(X ?? PY)) < m. Moreover, since Xj is the
constant map, E{J\jv CK' = EV(CK'/K'), and this space is simply connected.
Thus ? is a weak homotopy equivalence, by an obvious homology calculation.
376 28 Rational LS category and rational cone-length
Since ? V (CK' jK') has the homotopy type of a CW complex we conclude from
Lemma 28.2 that
cato X < cat X < cat (E V (CK'/K1))
< cat (E Ux C(X ? ? PY)) < m .
D
Corollary /// is as in Theorem 28.1 and X is a CW complex then cat/jj =
proof: Let cat/Q = m and let pm : Pm —» Yq be the mth Ganea fibration for
Yq. Then /q = pma for some continuous map ? : Xq —> Pm (Proposition 27.8).
In particular, ??,(?) <g> Q is injective and so
m > cato Pm > cato Xq = cat Xq
(Proposition 28.1). But also m = cat/Q < catXq by Lemma 27.1. ?
Example 1 Postnikov fibres.
Let X be a simply connected CW complex and suppose ??{?) = 0, i < r.
From Proposition 4.20 we obtain a fibration X -?*· ? (??(?), r) such that ???(?)
is the identity. If ? is a CW complex mapping by a weak homotopy equivalence
to the fibre of ? then the composite ? —>· X satisfies: ?^(/) is an isomorphism
for i > r + 1. In this way we obtain a sequence of maps
__^ ??+\ 9j^ , , . __^ ?3 _?^ ?2 _ ?
such that Xn+l is an ?-connected CW complex and ni(gn) is an isomorphism
for i > ? + 1. The Xn are called Postnikov fibres of X.
Notice that we can apply the Mapping theorem to this sequence to obtain
¦ · · < cat0 Xn <¦¦· < cat0 X2 < cat0 X .
In particular, if X has finite rational LS category, so do all its Postnikov fibres.
D
Example 2 Free loop spaces have infinite rational category.
Let X be a topological space. The free loop space of X is the space Xs of all
continuous maps S1 —> X (§0). We show that
• If X is two-connected and if H+(X;Q) ? 0 then
cato(X5 ) = oo .
Rational LS-Category 377
(Better results can be proved with a little more work.)
Indeed, let e e 51 be a basepoint. Evaluation at e defines a fibration ? :
Xs —> X whose fibre at a basepoint x0 € X is the loop space UX. The map
s : X —» Xs which associates to ? the constant loop 51 —> ? is a cross-section
for p, and it follows that the inclusions j : ?.? —> Xs and s : X —> Xs1 are
injective in homotopy.
Suppose cato(Xsl) = m < oo. Since X is 2-connected, ??' and „Ysl are
simply connected. Thus Theorem 28.6 asserts that if cato (Xs1) = m < oo,
cato X < m and cat0 ?? < m. Suppose ft : 52n;+1 —> X and ga : S2mi —> X
represent linearly independent elements in 7r„(X) <g> Q. Then, as in Proposi-
tion 16.7, ?/; ? Hgj : ?]?52??+1 ? ?5"'^ —> ?? is injective in ratio-
i j i j
nal homotopy, and so the category of this product is bounded by m. Since
H* (Q52ni+1;C) is a polynomial algebra (Example 1, §15(b)) it follows that
TTodd(-X) <8> Q = 0. and dim 7reVen(X) ® Q is finite. Now X has a Sullivan model
of the form AVeven and so its cohomology is a polynomial algebra, contradicting
cato X < oo. D
(d) Gottlieb groups.
Recall (Theorem 1.4) that any based topological space (X,x0) admits a weak
homotopy equivalence from a based CW complex (Y, yo), called a cellular model
for X. In particular an element a € ??(?) is representable by a unique based
homotopy class of continuous maps / : E",*) —> (Y,yo).
Definition a ? ??(?) is a Gottlieb element for X if (/, id) : Sn V Y —> Y
extends to a continuous map ?/ : Sn ? ? —i Y. (Note that this condition is
independent of the choices of / and of cellular model.)
Remark Gottlieb elements were introduced by Gottlieb in [67]; however, not
with this name!
Given a second Gottlieb element ? 6 ??(?) represented by g : Sr —> Y we
note that
restricts to (f,g, idY) in 5" V Sr V Y. More generally, if /f represents a Gottlieb
element in ???; (X) then (ft,..., fk, idy) : S V · · · V S"* V Y —> Y extends to
a continuous map
Sni x · · · x Snk x Y —> Y .
Next observe that the Gottlieb elements form a group. In fact if /, g :
En, *) —> (Y, y0) then [/] + [g] is represented by En, *) ? En V 5n, *) -^4
(Y, yo) as described in the proof of Lemma 13.6. Since (f,g,idy) extends to a
map S"xSnxr-> Y, \(ftg)j, idy) extends to 5" ? ?. The fact that the
inverse of a Gottlieb element is a Gottlieb element is obvious.
378 28 Rational LS category and rational cone-length
Definition The group of Gottlieb elements in ??(?) is called the nth Gottlieb
group of X and is denoted by Gn(X)· (It is an invariant of weak homotopy
type.)
If X is simply connected the group Gn(X^) of Gottlieb elements in Xq is
called the nth rational Gottlieb group of X and will be denoted by G®(X). -Note
that G^(X) is a rational subspace of ???{?) <8> Q and contains Gn(X) <g> Q. This
containment may be strict (see Exercise 1).
Recall from §21 (d) the definition of the rational homotopy Lie algebra Lx =
n»(UX) g> <Q> = 7t,(UXq). By definition, the Lie bracket in Lx corresponds
up to sign to the Whitehead product in ?,(?) <g> Q under the isomorphism
(Lx). ~?.+?(
Proposition 28.7 If a € (Lx)n corresponds to a Gottlieb element inirn+i(X)<g>
Q then
[a,?}=0, ?eLx.
proof: Let a € ??+1 (X) <8> Q be the Gottlieb element corresponding to a and
let a be represented by a map / : (S"+1, *) —? {?, *). Without loss of generality
assume X is a rational CW complex. Then for any g : (Sk, *) —? (?, *) the map
(f,9) '¦ Sn+1 V 5* —> X extends to a map Sn+1 ? Sh —> X, which shows that
the Whitehead product of ? = [/] and [g] is zero (§13(d)). D
Example 1 G-spaces.
Suppose a topological monoid G acts on a topological space X with basepoint
#?· Restricting the action to {#?} x G defines a continuous map j : G —> X
and, essentially by definition,
lmicn(j)CGn(X) ·
In particular, nn(G) = Gn(G). ?
Example 2 The holonomy fibration.
Suppose / : X —> Y is a continuous map and, as in §l(c) construct the
holonomy fibration ? ? ??? —? X with fibre ?,?. Since the inclusion i : ?.? —?
X xy PY of the fibre extends to an action of UY it follows as in Example 1 that
Imirn(i) CGn(X xY PY) , n>l.
Now suppose / is itself a Serre fibration with fibre inclusion j : F —)¦ X. Then
there is a weak homotopy equivalence between ? ? ? PY and F which identifies
1????(?) with ker7rn(i). It follows that
keritnU) CGn(F) , n>l. ?
Proposition 28.8 Suppose X is a simply connected topological space of finite
rational LS category. Then
Rational LS-Category 379
(i) G®(X) is concentrated in odd degrees, and
(ii) dimG®(X) < cato A'.
proof: (i) We may assume A^ itself is a simply connected rational CW complex,
and hence that Gm(X) = G®(X)· Suppose first that / : S2k —> X represents a
non-zero Gottlieb element, and that X is BA; - l)-connected. By Theorem 4.19,
¦#2Jfc(A';<Q>) = 7it(A") ® Q = ^-(AT)· Thus there is a cohomology class w €
H-k(X; Q) such that H*(f)w ? 0. Extend (/,...,/, idx) to a map ? : S2k ?
• · · ? S7k ? ? —? X and observe that ?*{?)?? = Hm(f)w <g> 1 <g> · · · <g> 1 +
• · · + 1 g» · · · g» H'm(f)w <g>l + l<g>---<g>l<g>i/;. If we have used ?-factors 5-fc
then ?*{?)??? = {?*{?)??)? ? 0. It follows that ?? ^ 0 for all ? and hence
cat0X = oo.
Now suppose X is only simply connected, and let X2k —> X be its 2kth
Postnikov fibre. If / : S'k —> A' represents a non-zero element of Gok{X) then
we may assume / : S2k —l· X~k. Apply the argument of Lemma 1.5 to the
diagram
s2kvx2k —{Lid) ' x2k
s-k ? ? ?
to fill in the dotted arrow making the upper triangle commute. This identifies /
as representing a non-zero element of G2k(X2k)-
But now the Mapping theorem 28.6 gives cat0 X > cato X2k = oo.
(ii) Again we may suppose A' is a simply connected rational CW com-
plex. Thus G®(X) = Gm(X)· If Qi,...,Qr are linearly independent elements
of Gm(X) then; as at the start of this topic we can extend representatives
fi : Sn: —? ? to a map
? : Sni x · · · x 5n- x X —> X .
Let g be the restriction of ? to Sni ? ·· · ? Sn". Then by (i) each n; is odd
and so ??.E";) <g> Q = 7rn,.Eni) <S> Q = Q (Example 1, §15(d)). It follows that
?*{?) <8> Q is injective and so the Mapping theorem 28.6 asserts that cato A" >
catotS ? · · · ? S·). But the rational cup length of Sni x · · · ? Snr is r and so
catoEni ? · · · ? Sn') > r. Thus cato Jf > dimG^(A'). ?
CorolLary Suppose j : F —l· X is the fibre inclusion of a Serre fibration
X —> Y, and that F is simply connected. If cat0 F < oo then ker 7r„(y) ® Q is
concentrated in odd degrees and dim (ker ??, (j) <g> Q) < cat0 F.
proof: By Example 2, above, ker7r,(i) ® Q C G,(F) ® Q C G®(F). ?
380 28 Rational LS category and rational cone-length
Example 3 Loop spaces.
Suppose ?? is a simply connected loop space. We show that
cat0 ?? < oo <=» ?*(?.?\ Q) = AV , with V = Vodd and dim V < oo .
In fact if cat0 UY < oo then ?,(?.?) <g> Q = G,(UY) <g> Q (Example 1) and
hence is finite dimensional and concentrated in odd degrees. Thus ?,(?) <g>Q is
finite dimensional and §16(b) asserts that UY has a Sullivan model of the form
(AV, 0) with V = ?, (UY) ? Q. "
Conversely if H*(UY;Q) = AV as above then (AF,0) is a Sullivan model
for ?.? and ?,(??) (8>Q is a finite dimensional vector space concentrated in odd
degrees. If fi : S2n;+1 —? UY represent a basis then multiplication in QY defines
?
a rational homotopy equivalence ? 52??+1 —> CIY and so cato ?? = r < oo. ?
Exercises
1. Prove that if ? € GnX, then [?, ?] = 0 for any ? e nkX.
2. Let X = S3 V (Vn>45n) Un>4 (on[s3,S"}Dn+3). Prove that ?3(?) = ?,
G3(X) = 0, and G^(X) = Q.
3. Let / : Sn -> X be a continuous map such that [/] € Gn(X), and
ifn(/;Z)([Sn]) ? 0. Prove that X has the same homotopy type as ? ? Sn.
4. Let / : X -> F be a continuous map, and suppose that ?,(/) <8>Q is injective.
Prove that cato/ = cato-X"·
5. Let f ¦ X —> Y be a continuous map such that H*(f\Q) is injective. Prove
that eo(JO > eo(Y).
29 LS category of Sullivan algebras
In this section the ground ring is an arbitrary field k of characteristic zero.
Let X be a simply connected topological space with rational homology of finite
type. Then the rational homotopy type of X is completely determined by its
minimal Sullivan model (§12, §17) or by any Lie model (§24). It is thus not un-
expected that we should be able to compute cl0 JY, cat0 X and eQX directly from
these models. Recall also that a commutative model for X is any commutative
cochain algebra connected to Apl(X) by a chain of quasi-isomorphisms (§10).
Thus (A, d) is a commutative model for X if and only if its minimal Sullivan
model coincides with that of X.
Now we introduce the following
Definition Suppose A = A-0 is a graded algebra with A0 = Ic. The prod-
uct length of A, denoted by nil A, is the greatest integer ? (or oo) such that
.4+ A+ ? 0 (? factors).
Then we prove (§29(a)) a result of Cornea's [40]:
• clo X < ? 4=> nil A < ? for some rational com-
mutative model (A,d) of X.
Note that H(A,d) = H*(X;.Q) and so the product length of ? (A) is just the
cup length c(X;Q) as defined in §27(f). Thus cl0 X = ? corresponds to product
length ? in a suitable cochain algebra model, while c(JY;Q) = ? corresponds to
product length ? in the cohomology algebra.
Next, suppose (AV,d) is any Sullivan algebra (§12), and m > 1. Recall that
(AV,'d) is filtered by ideals (A>mV, d) of elements of word length > m. The
surjections gm : (AV, d) —> (AV/A>mV,d) extend to relative Sullivan models of
the form
(AV,d) —^-* (AV®AZ(m),d)
(AV/A>mV,d) ,
as described in §14(a).
Definition The LS category, cat(AV,d), is the least integer m (or oo) such
that there is a cochain algebra morphism nm .· (AV ® AZ(m),d) —> (AV, d)
such that nmXm = idAv-
The Toomer invariant, e(AV,d), is the least integer r (or oo) such that ?(??)
is injective.
In §29(b) we then establish
382 29 LS category of Sullivan algebras
• // (AV, d) is a rational Sullivan model for X then
cato A' = cat(AV. d) and e0X = e(AV, d) .
We shall deduce this as a corollary of Cornea's theorem, although the result itself
is older (with a different proof). Then we shall use this to give easy examples in
which eoX < cato A". Notice that these results indicate a close relation between
the topological filtration P0X c · · · C PmX C · · · and the algebraic filtration
AV D A+V D---D A>mV D · · -.
The second result above reduces the computation of cato X to the existence
(or non-existence) of retractions (AV <g> AZ(m),d) —> (AV,d). Constructing
such morphisms, however, is not easy, because they are required to be algebra
morphisms. Fortunately, a magnificent theorem of Hess [90] about any Sullivan
algebra over k comes to our rescue (§29(e)):
• cat(AV, d) is the least integer m such that there is a morphism of (AV,d)-
modules, irm : (AV <g> AZ(m),d) —> (AV,d), such that Trm\m = id\v-
Finally, in §29(g) we shall extend the notion of e(AV, d) to e(M,d), for any
(AV, d)-module (M,d). In particular, we may consider the module (AV,d)i =
Homft(AV,*) with (a · f)(b) = (-l)de*ade*ff(ab), a,b G AV, / G (AV)*. If V
is a graded vector space of finite type we use Hess's theorem to prove the recent
result of Felix, Halperin and Lemaire [55]:
This section is organized into the following topics:
(a) The rational cone-length of spaces and the product length of models.
(b) The LS category of a Sullivan algebra.
(c) The mapping theorem for Sullivan algebras.
(d) Gottlieb elements.
(e) Hess' theorem.
(f) The model of (AV,d) —> (AV/A>mV,d).
(g) The Milnor-Moore spectral sequence and Ginsburg's theorem,
(h) The invariants meat and e for (AV, d)-modules.
(a) The rational cone-length of spaces and the product length of mod
els.
Our central result provides an algebraic description .of cone-length. It reads
Theorem 29.1 Let Ik = Q. The following conditions are equivalent for
simply connected topological space X with rational homology of finite type:
Rational LS-Category 383
(i) do A' < ?.
(ii) rii\A<n for some commutative model (A, d) for X with A0 — Q.
(Hi) X has a free Lie model (hy,d) with an increasing filtration 0 = V@) C
V(l) C - - - C V(n) = V such that for each i, d : V(i) —> Ly (,_!).
(iv) There is a rational homotopy equivalence ? —y X from a spherical n-cone
whose constituent spaces are wedges of spheres with finitely many spheres
in each dimension.
The proof of the theorem requires one small technicality.
Lemma 29.2 Suppose (A,d) is a commutative cochain algebra (over any k of
characteristic zero) such that A° = Ik, H1(A) — 0 and each H'(A) has finite
dimension. Then there is a subcochain algebra (B, d) such that; Bl = 0, each
Bl is finite dimensional and d : B+ —> B+ · B+.
proof: Choose A C A so that A1 = 0, ?2 ? ?(??) = A2 and A* = A\ i > 3.
Then (A,d) ^> (A, d) and so we may suppose A1 — 0.
Suppose next that A' is finite dimensional for i < n, and let A be the sub-
algebra generated by A<n and (Im<2)n. Then A is a sub cochain algebra and
.each A1 is finite dimensional. Now write .4" = d~1(An+1) ? Un and choose
C CAso that (i) ? = A1, i < n, (ii) Cn = ??(??+1), (iii) Cn+l D An+l and
Cn+1 ? dUn = An+l and (iv) Cl = Ai,i>n + 2. Then (C, d) is a sub cochain
algebra including quasi-isomorphically in (A, d) and Cl is finite dimensional,
i < n.
We now construct a decreasing sequence of quasi-isomorphic inclusions of sub
cochain algebras A D .4B) D .4C) D ¦¦¦ as follows: if A(k) is constructed so
that A'(k) is finite dimensional, i < k, then A(k + 1) is a subcochain algebra
including quasi-isomorphically in A(k) such that Al(k + 1) = Al(k), i < k and
such that among all these Ak+i(k + l) has minimal dimension (necessarily finite
by the argument above). Set ? = ?^(^) and note that Bl = Al(k), i < k,
k
so that (B, d) -=> (A,d). Note as well that for any proper sub cochain algebra
(B, d) C (B, d) the inclusion is not a quasi-isomorphism.
Let ? : ? —> k®B+ /B+ · B+ be the projection and let Q{d) be the differential
in B+/B+ -B+. If kerQ(d) - ? ? imQ(d) then C^ © ii) is a sub cochain
algebra including quasi-isomorphically in B. Thus ?~*(& ? ?) = B and H =
B+/B+-B+;\.e.,Q(d) = 0. ?
proof of Theorem 29.1: (i) => (ii). If cl0 X = 1 then ? ~ ?? for a well-
based space Y, and the desired conclusion is exactly Proposition 13.9. For ? > 1
we may suppose X = Y U/ CZ where clo Y < ? — 1 and ? and Y are path
connected. Let my : (AVy,d) —> Apl(Y) be a Sullivan model and extend
384 29 LS category of Sullivan algebras
ApL(f)m,Y to a Sullivan model mz : (AVy ? AV,d) -=> APL(Z). Then the
inclusion A : AVy —> AVy <g> AV is a Sullivan representative for /.
As usual, let A(i,eft) be the free commutative cochain algebra on genera-
tors t and dt of degrees zero and one and let 6\ : A(i, dt) —l· Q be the aug-
mentation sending t >-> 1. The morphisms —> -f—— define a fibre product
AVy ??vy®av (Q ? [AVV ® AV ® ?+(?, dt)}), and Proposition 13.8 identifies this
as a commutative model for X.
By induction there is a quasi-isomorphism of commutative cochain algebras
(AVY,d) —> (B,d), where 5° = Q and m\B < ? - 1. Apply 5 <8>??? - to
obtain a quasi-isomorphism (AVy- ® AV,d) ^i (B <g> AV,d) as described in
Lemma 14.2. This identifies ? xb®av (Q®[B ® AV ® A+(t,dt)]) as a com-
mutative model for X. This contains (A,d) — ? ?#®?? (Q@[B+®AV<g>
A+(t,dt)j ? (AV <8> dt)) as a sub cochain algebra. Since the inclusion of dt in
A+(i, dt) ? ker ei is a quasi-isomorphism, so is the inclusion of (A, d) in the fibre
product. Thus (.4, d) is a commutative model for X satisfying nil A < n.
(ii) => (in). If (ii) holds we may use Lemma 29.2 to find a commutative model
(B, d) for X such that B° = Q, B1 = 0, each Bl is finite dimensional, d : B+ —>
B+ -B+ and also nil ? < ?. Then ?(?M) is a Lie model for X (§23(a)). Now
C(B,d) =U- withV = s-1Hom(B+,Q).'Put/(fc) = B+ B+ (fc+1 factors)
and dualize the sequence
0 *- 5+//(I) <— B+/IB) < <— B+/I(n) = 5+
to obtain a filtration of V. The facts that 5+ -I(k) C /(& + 1) and d : I(k) —>
I(k + 1) imply that the filtration on V has the stated properties, as follows
immediately from the definition of the differential in C{B,d)-
(iii) => (iv). We may clearly suppose X is a CW complex. It follows at once from
Theorem 24.7 that (Lv, d) is the Lie model of a space of the form P. This means
that X and ? have the same rational homotopy type, and so there is a weak
homotopy equivalence Xq -=> Pq. Now apply Lemma 28.4 to the composite
X -+Pq.
(iv) => (i). This is obvious. D
(b) The LS category of a Sullivan algebra.
We begin by proving
Proposition 29.3
(i) Let (AV, d) be a Sullivan algebra. Then cat(AVr, d) < m if and only if there
Rational LS-Category
is a diagram of commutative cochain algebras
(AV, d)
(A,d)
(C,d)
385
(B,d)
in which ?a is a quasi-isomorphism and nil ? < m.
(ii) If(AV, d) and (AW, d) are quasi-isomorphic Sullivan algebras then cat(AV, d)
cat(AW,d).
proof: (i) Recall the diagram at the start of this section. If cat(AV, d) < m,
that diagram, together with the retraction (AV ® AZ(m),d) —i (AV,d) satisfies
the Proposition. Conversely, given such a diagram note that ?? factors to yield
7 : (AV/A>mV,d) —> (B,d) with jgm = ??. In the diagram
(AV,d)
(AV®AZ(m),d)
(A,d)
(B,d)
we may extend ? to a morphism ? : (AV <8> AZ(m),d) -
?? ~ 7Cmrel (AV,d). Finally, in the commutative diagram
(A, d) such that
(AV, d)
(AV ®AZ(m),d)
(AV,d)
(C,d)
we may extend id\v to a retraction irm : (AV g> AZ(m),d) —> (AV,d).
(ii) This is a trivial consequence of (i). ?
Corollary 1 Suppose for some ? > r > 1 that the non-zero elements of
H+(AV,d) are concentrated in degrees i with r < i < n. Then
ca.t(AV,d) < n/r .
386 29 LS category of Sullivan algebras
proof: It is easy to construct a quasi-isomorphism (AV, d) -=» (B,d) in which
B+ is concentrated in degrees i, r < i < n. Then nil ? < n/r. Apply the
Proposition. D
Corollary 2 m\H(AV,d) < e(AV,d) < cat(AV,d) < niL4 for any commuta-
tive model (A, d) of(AV.id). In particular, if H{AV, d) is finite dimensional then
cat(AK,d) < max{i|iT(AV, d)^0}.
proof: The inequalities are immediate, the first two from the definitions and
the third from the Proposition. Finally, if H(AV, d) is zero in degrees > ? then
H(I) = 0 where / is the ideal defined by / = (AV)>n ? Un, with Un chosen so
(AV)n = Un ® (kerd)n. Thus (AV,d) —> (AV/I,d) is a quasi-isomorphism and
cat(AV, d) < nil(AV/7) < n. D
Next we establish
Proposition 29.4 Suppose (AV, d) is a rational Sullivan model for a simply
connected space X with rational homology of finite type. Then
cat(AV, d) = cat0 X and e(AV, d) = e0X .
proof: We may suppose X is a rational CW complex, so that cat0 X = cat X
(Proposition 28.1). Recall the quasi-isomorphism Cm : (AV <g> AZ(m),d) -=>
(AF/A>ml/,d) defined at the start of this section. The Sullivan algebra (AF ®
AZ(m),d) is a Sullivan model for the realization Y = \AV ® AZ(m),d\, as we
showed in Theorem 17.10. Thus (AV/A>mF,d) is a commutative model for
Y, which implies that clo^) < m (Theorem 29.1). Since Y is a rational CW
complex, Y has the homotopy type of an m-cone (Proposition 28.3).
Suppose cat(AV,d) = m. Then there is a morphism 7rm : (AV®AZ(m),d) —>
(AV, d) such that nmXm = id. Thus \Xm\ \irm\ = id\Av,d\ and |AF,d| is a retract of
Y. Since |AV, d\ ~Q X (Theorem 17.12) it follows that cat0 X < cat |AV, d\ < m.
Conversely, if cat0 X = m then cat X — m and X is a homotopy retract of
an m-cone ? (Theorem 27.10). Let (AV,d) A (AVP,d) ? (AV,d) be Sullivan
representatives respectively for the retraction and the inclusion. Then ?? is
a quasi-isomorphism and hence an isomorphism (Theorem 14.11). Moreover,
Theorem 29.1 provides a quasi-isomorphism ? : (AVp,d) -=> (A,d) with nil.4 <
m. Thus ca.t(AV,d) < m (Proposition 29.3).
Finally, since e0X = e(X;Q) this is the least integer, r, such that there
is a continuous map / of X into an r-cone such that Hr(f;Q) is injective
(Proposition 28.1). Now a simplified version of the argument above shows that
e(AV,d) = e0X. ?
Corollary Suppose (AV, d) is any rational Sullivan algebra with V = {V1} .>0
a graded vector space of finite type. Then the spatial realization (§17) \AV,d\ is
Rational LS-Category 387
? rational space satisfying
cat IA V, d\ =cat(AV,d) .
proof: According to Theorem 17.10, |AK, d\ is a simply connected rational
topological space with (AV, d) as a Sullivan model. Thus cat |AV, d\ = cat0 |AV, d\ ¦¦
cat(AV, d), by Proposition 28.1 and Proposition 29.4 D
Example 1 A space X satisfying cqX < e0X.
Let X be a simply connected space with Sullivan model (A(x,y, z),d) where
dega: = 3 = degy, dx = dy = 0 and dz = xy. Then the cohomology algebra
H*(X;Q) has 1, [x], [y], [xz], [yz] and [^[yz] as basis, and so
c0X = 2 .
On the other hand, nil (A(x, y,z)) = 3 and so 3 > cloA' > cat0X > e0X.
Finally, xyz G A3(x,y,z) represents a non-trivial cohomology class, so e0X > 3.
Thus
e0X = cat0 X = cl0 X = 3 . D
Example 2 (Lemaire-Sigrist [111]) A space X satisfying e0X < cat0A'.
Consider the commutative cochain algebra (A, d) given by
A = ?(?, y, t)/(x4, xy, xt) , dx = dy — 0, dt = ?3 ,
with dega; = 2, degy = 3 and degi = 5. Evidently
nil(A,d) =3 .
Thus if X is a simply connected space with (.4, d) as commutative model then
cato X < cl0 X = 3 .
A vector space basis for A+ is given by x,x2,x3,y,t,yt and so a vector space
basis for H+{A) is given by [x], [x]2, [y], [yi\. In particular. H(A) is concentrated
in degrees < 7. A minimal Sullivan model (AV, d) for (A, d) has the form m :
? (?, y,z,t,...) —> A with mx = x, my = y, mz = 0, rat = t and dz = xy; the
remaining generators all have degree at least 7. In particular A^3V = hzx2 ®
(A-3F)>?. Since zx2 is not a cocycle no cohomology class can be represented
by a cocycle in A-3V:
= e(AV,d) = 2.
Finally, we show that cat(AV, d) > 2, thereby establishing
do (JO = cat0 (?') = cat(AV, d) = 3 .
388 29 LS category of Sullivan algebras
Thus in particular, eo{X) < cato (A'). In fact, let ? : (AV,d) —> (AV/A>2V,d)
be the projection. If cat(AF, d) < 2 then there is a morphism of graded algebras
? : H (AV/A>2V) —)¦ if(AF) such that ????(?) = id. Now t becomes a cocycle
in AF/A>2K and so we would have [yt+x2z] ^ [y][t] hA 0. Since [yt+x2z] »—>
[yi] in #(,4), [yi + ?2 ?) ? 0 and this contradiction proves cat(AV, d) > 2. ?
Example 3 {Dupont [45]) A space X satisfying cato X < clo X.
Let (L, d) be the free differential graded Lie algebra L(a, b, x, y) with deg a = 3,
dege = 5, deg a; = 7, degy = 11 and da = db — 0, dx = [a,a], dy = [a,x] + [b,b].
Let (L', d) = (L(a', 6', x', y'M) be a copy of L and extend L(a, 6, x, y, a', 6', x', y')
to the free dgl L(a, 6, a;, y, a', b', x', y', a", u, u', 6", w) by requiring
da" = [a,a'}, du = [a,6'], du' = [a',b], db" = [b,b'}
and
dv = [[a, [b,b]}, 4[a',y'] + [?',?']] - 7 ,
where 7 G L(a,b,a' ,b',a",u,u',b") has bracket length 5 and satisfies dy =
4[[a,[b,b}},[a',[b',b'}]].
In [45] Dupont shows that if X is a simply connected space with Lie model
(L, d) then
clo X = 4 and cat0 X = 3 .
Since the argument is lengthy and difficult, we do not reproduce it here. D
Example 4 Formal spaces.
Let X be a simply connected topological space with rational homology of finite
type. If X is formal then
co(X) = eo(Ar) = cato X = cl0 X .
In fact, since X is formal H*(X) is a commutative model for X. Thus co(X) =
nil if* (A') > clo X, by Theorem 29.1. The reverse inequalities are established in
Theorem 28.5. D
Example 5 Coformal spaces.
Recall that a simply connected topological space X with rational homology of
finite type is coformal if it has a Lie model of the form (L, 0) — Example 7, §24
(f). Equivalently X has a purely quadratic Sullivan model (AV,d\). We show
for coformal spaces that
e0p0 =cato(X) = doX .
In fact, suppose eo(A') = r. Choose a vector space complement S for kerdi
in ArV. Then / = S @ A>rF is an acyclic ideal and (AV/I, d) is a commutative
model for X. Since m\{AV/I,d) = r we have (Theorem 29.1) that r > c\0X.
The reverse inequalities are always true, as in Example 1. ?
Rational LS-Category 389
Example 6 Minimal Sullivan algebras (AV, d) with V = yodd ??? dim y < oo.
If (AF, d) is as in the title of the example, let r = dim F. Then ? F = ? A'F,
dimArF = 1 and the elements in ArV are cocycles and not coboundaries. Thus
e(AV,d)=r = nil(AV,d) and hence e(\V,d) = cat(AF,d) = r. D
Example 7 (AV, d) = A(a, 6, a;, y, z) with dx = a2, dy = b2 and dz = ab.
In this example we take dega = 2, degb = 2, but any even degrees would do.
Hence (AV, d) -=> Aa/a2 ® Ab/b2 ® Az, and this commutative model (v4,d) has
nil A = 3. A non trivial cohomology class is represented by ab ? — xb2 and so
e(AV, d) > 3. It follows that e(AV, d) = cat(AV, d) = 3. ?
(c) The mapping theorem for Sullivan algebras.
The Mapping theorem 2S.6 for topological spaces (§28(c)) has an analogue for
Sullivan algebras. In the case Jk = Q and the spaces and algebras have finite
dimensional cohomology in each degree Theorem 28.6 follows from the theorem
for Sullivan algebras, which reads:
Theorem 29.5 Suppose ? : (AV, d) —> (AW, d) is a morphism of minimal
Sullivan algebras with V = {Vl}i>2 and W = {H/Z}i>2.
(i) If ? is surjective then cat(AF.d) > cat(AW,d).
(ii) If there are r elements of odd degree, W\,..., wr € W such that AW =
Im ? · A(wi ,...,wr) then cat(AF, d) + r > cat (AW, d).
proof: (i) Extend ? to a quasi-isomorphism ? : (AV <g> AZ, d) —> (AH^.d)
from a minimal relative Sullivan algebra, and let ? : (AV <g> AZ, d) —> (AZ, d)
be the surjection obtained by setting V = 0. Choose a quasi-isomorphism ? :
(AW,d) ^ (AV ®AZ,d) such that ?? = id (Lemma 12.4). We first establish
• The differential d in AZ is zero. B9.6)
and
• ?(?+\?) CA+V&AZ. B9.7)
Indeed let (AV<8)AV, D) -^ Ik be a minimal Sullivan model for the augmenta-
tion ? F —> 3z. Thus (§14(a)) ? extends to a quasi-isomorphism (AF® AF)®av
(AV ® AZ) A (AZ,d). Moreover ??®? : (AV ® AV) ®av (AV ® AZ)_-=>
(AV ® AV) ®av APV and so (AZ, d) ~ AW ®av (AV ® AV) = (AW ® AV, ?).
The linear part, ?0, of ? is the map Q(ip)D0 : V —> W, where Q(ip) is the linear
part of ?.
Now (AF ® AF, D) is contractible, because it is a Sullh'an algebra quasi-
isomorphic to k (Theorem 14.9). Thus Do : V -=> V and so ?0 is surjective.
Write V = Fo ? Vi with Fo = kerJ0- Then recall (Example of §14(&)) that
390
29 LS category of Sullivan algebras
the quotient differential in AV is zero. Thus a composite morphism 7 : (AW <S>
??, ?) —)¦ (AV, 0) —> (AV0,0) is defined by setting first W7 and then Fi to zero.
By construction H(Q(j)) is an isomorphism and hence 7 is a quasi-isomorphism
(Proposition 14.13). This shows that (AVo,0) is a minimal Sullivan model for
(AW ® AV,6). It follows that (AZ,d) 9* (AFo,0), which proves B9.6).
For the proof of B9.7) let ? : (AV,d) —> (AV®AZ,d) be the inclusion. Then
?? = ? = ??? and so ? ~ ?/></? (Proposition 12.9). Thus ?? ~ ?rcy</? : (AV, d) —>
(AZ, 0). But ?? is the augmentation in AV and so the Example in §12(b) asserts
that g\ = ???. In other words, gip(A+W) = ???(?+\?) = 0, which proves
B9.7).
Finally, we suppose cat(AV,d) = m and deduce that cat(AW,d) < m. Let
(AK, d) - Am > A V ® AZ(m)
(AV, d)
AV/A>mV,d)
be as described at the start of the section. Apply —
augmentation ideals of this diagram to obtain
(\AV]+®AZ,d) ({AV ® AZ(m)}+ ® AZ,d)
(AV ® ??, d) to the
([AV}+®AZ,d
([AV/A>mV]+®AZ,d),
where the vertical arrow is a quasi-isomorphism because (AV®AZ, d) is (AV, d)-
semifree (Lemma 14.1 and Proposition 6.7). Now B9.7) states that ? factors as
(AW, d) —> (Jb ? [AV]+ ® AZ, d) —> (AV <g> AZ, d). Thus it also factors as
(AW,d) (Ik ®{AV ® AZ(m)}+ ® AZ,d) (AV®AZ,d)
(? ? [AV/A>m V]+ «8» AZ, d)
Since the lower cochain algebra has product length m this is exactly the situation
envisaged in Proposition 29.3, which therefore asserts that c&t(AW,d) < m.
(ii) This is proved by induction on r, the case r = 0 being precisely
assertion (i). Assume deg^i < · · · < degu;r. Set degun = p and put (AZ, d) =
(AW<p,d)®{AV<p,d)(AV,d) = (AW<P®AV^P,d). Then ? factors as (AV,d) ?
(AZ, d) —> (AW,d) and ? is surjective. It follows that ????' · A(w\,... ,wT) =
AW and (by assertion (i)) that cat(AV,d) > cat(AZ,d).
Rational LS-Category. 391
Thus it is sufficient to prove assertion (ii) for ?'; i.e., without loss of generality
we may and do assume that ? is an isomorphism from AV<P to AW<P. Since
V and W are concentrated in degrees > 2, and since (AW,d) is minimal, dwx
is a cocycle in AM/<P. Extend ? to a morphism ? : (AV ® Au,d) —> (AW, d)
by setting du = ip~1dwi and ??. = w\. By induction, cat(AU',d) < cat(AV ®
Au, d) + r - 1. It remains to prove cat(AV ® Au, d) < cat(AV, d) + 1.
For this, apply - ®av (AV ® Au,d) to the diagram of Proposition 29.3(i),
with nil ? = cat(AV, d). Now ? ®av (AV ® Au) = ? ® Au. Since degu is odd,
nil (B ®av7 (AV ® Au)) = nil ? + 1. Since — ®av" (AV & Au, d) preserves quasi-
isomorphisms it follows immediately from Proposition 29.3(i) that cat(AV ®
Au, d) < nil ? + 1 < cat(AV, d) + l. D
Example 1 e ((AV,d) ® (AW, d)) = e(AK, d) + e(A\V, d).
Let (AV,d) and (AH7, d) be any minimal Sullivan algebras. Then e(AV, d) is
the least integer r such that (AV, d) —> (AV/A>rV, d) is injective in cohomology.
Thus it is the greatest integer r such that A V-r contains a cocycle ? representing
a non-zero class in H(AV).
Let s = e(AW, d) and w G A-SIV be a cocycle representing a non-zero class in
H (AW). Then v®w G A^T+S(V®W) and [v®w] ? 0, so e ((AV, d) ® (AIV, d)) >
r + s.
Conversely the surjection AV® AW —> AV/A>rV® AW/A>SW is injective in
cohomology, and its kernel contains A>r+s(V®W). Thus e ((AV, d) ® (AW, d)) <
r + s; i.e. e ((AV, d) ® (AW, cQ) = e(AV, d) + e(AW, d). ?
Example 2 cat0 ?' — e0 X can be arbitrarily large.
Let X be the space of Example 2, §29(b). Its minimal Sullivan model (AV, d)
satisfies cat(AV, d) = 3 and e(AV, d) = 2, and has the form A(x,y, z,t,u,v,...)
with dx = dy = 0, dz = xy, dt = x3, du = zy, dv = z2 - 2ux and with dega; = 2,
degy = 3, degz = 4, degi = 5, degu = 6 and degi> = 7. Set u and y to zero to
define a surjection
(A(x, y, z, t, u, v),d) —> (A(x, z, t, v), d)
with dx = dz = 0, dt = x3 and dv = z2; denote this quotient Sullivan algebra
by (AW,d). Then H(AW,d) is concentrated in degrees < 8 while the remaining
elements of V have degrees at least 8. It follows that the surjection above extends
to a surjective morphism ? : (AV,d) —> (AW,d). Taking tensor products we
obtain surjective morphisms
®?? : (AV,d)9n —» (AW,d)®n .
The cocycle zx2 in A3 W represents a non trivial cohomology class. Thus
e(AW,d) > 3 and e((AW,d)®n) > 3n (Example 1). Now apply the Mapping
theorem 29.5 to obtain
cat(AV,d)®n > ca.t(AW,d)®n > e(AW,d) > 3n .
392 29 LS category of Sullivan algebras
On the other hand, again by Example 1,
e(A V, d)®n = ? e(AV, d)=2n .
Thus if X" = ?' x · · · x ?' (n factors),
cato(A'n) > 3n and eo(Xn) = In .
(In fact, it is not too hard to show that cato(A'n) = 3n.) D
(d) Gottlieb elements.
A Gottlieb element of degree ? for a minimal Sullivan algebra (AV, d) is a
linear map / : Vn —> k that extends to a derivation ? of (AV,d), that is, a
derivation of AV satisfying ?? = (~1)???. The Gottlieb elements form a graded
subspace G.(AV,d) C Hom(V, Jfe).
Now suppose X is a simply connected topological space with rational homology
of finite type. Recall that the choice of a rational minimal Sullivan model m :
(AV,d) -=» Apl(X) determines an isomorphism
?? : V A HomQ (tt»(A'q),Q) ,
as follows from Theorem 15.11.
Proposition 29.8 The dual of ?? restricts to an isomorphism (§28(d))
(i) G.(AV,d) 4
(it) If (AW, d) is any minimal Sullivan algebra with W = {VFP}P>2 and ca.t(AW, a
< m then G» (AH7, d) is concentrated in odd degrees, and dim G, (AW, d) <
proof: (i) Write H*(Sn) - h @ he, where e G Hn{Sn) is dual to the funda-
mental class of 5". Any continuous map g : Sn ? Xq —> Xq is represented by a
morphism ? : (AV, d) —> H*(Sn) ® (AV,d) and conversely, any such morphism
represents a continuous map (Theorem 17.15). The maps g such that g
correspond to the morphisms ? of the form
id
in which case ? is automatically a derivation of degree —? in (AV, d).
Thus / : Vn —> Q corresponds to a Gottlieb element of Xq if and only if
f?Gn(AV,d).
(ii) Suppose 0^/6 Gok(AW,d), and extend it to a derivation ? of
(AW,d). Then ? = 0 in AW<lk and so dividing by A+W<2k · AW we obtain a
derivation ? in (AW-2k,d) restricting to / in W2k. Choose w e W'2k so that
Rational LS-Category 393
O(w) = f(w) = 1. Clearly dw_= 0 and Ok(wk) = kl Thus wk cannot be a
coboundary and m\H(AW-2k,d) = oo. Now apply the Mapping theorem 29.5
to conclude ca.t(AW,d) > cat (AW^2k,d) > nil H (AW^'2k, J) = oo.
On the other hand if 0 ? f G G2n+\(AW,d), extend / to a derivation ? of
(AW,d) and define a morphism ? : (AW,d) —> (Au,0) <g> (AW,d) of Sullivan
algebras by ? : ? ?—> 1 <g> ? + u ® #? (here degu = 2n + 1). If </?!,...,</?r
correspond to Gottlieb elements /i, ·.., /r of odd degree then form the composite
morphism
0- ..oVl :(AW,d) —>(A(ui,...,ur),0)®(AW,d) —> (A(Ul,... ,ur),0)
If the fi are linearly independent then this morphism is surjective and so
cat(At-F, d) > cat (A(«i,..., ur), 0) = r. D
Example 1 .4 non-trivial Gottlieb element.
Let (j4, d) be the commutative cochain algebra defined by A = A(a, b, x, y) /abxy
with dega = dego = dega; = 3 and dy = abx. The Sullivan model for (A,d) has
the form (AV,d) — A(a,b,x,y,z,...) with dz = abxy. We show that the map
f : y 1—> 1 is ? Gottlieb element for (AV, d).
In fact extend / to a derivation ? of (AV, d) by first setting ?? = yx. Then
note that elements of higher degree in V have degree at least 15, while H(AV, d)
is concentrated in degrees < 11. Thus ? extends automatically to the rest of V
so as to satisfy ?? + ?? = 0.
On the other hand, suppose (AV, d) is a Sullivan model for a simply connected
topological space X and let X —> Y be any fibration with fibre S5. It follows
from §15(a) and a simple calculation that the inclusion S5 —> X is zero in
rational homotopy. In particular the Gottlieb element / does not arise as the
fibre of an S5-fibration. D
(e) Hess' theorem.
Fix a minimal Sullivan algebra (AV,d) such that V = {Vi}i>2 an<^ fix an
integer n. Simplify the diagram in the introduction to
(AV,d)
in particular ? is a minimal Sullivan model for ?. Our goal is
Theorem 29.9 (Hess — [90]) Assume there is a morphism
?:(??®??,?) —>(AV,d)
394 29 LS category of Sullivan algebras
of (AV,d)-modules such that ?? = id. Then there is a morphism ?' : (AV <g>
AZ,d) —> (AV, d) of cochain algebras such that ?'? = id; i.e.,
cat(AV,d) < m .
Remark Theorem 29.9 holds even if V1 ? 0 and is so stated and proved in
[90]. We limit ourselves to the case V1 = 0 because the proof is then simpler. D
The proof of Theorem 29.9 requires a technical result about the structure of
(AV®AZ, d) which we state as Proposition 29.10 immediately below. The proof,
however, will be deferred to the next topic as only the statement is required for
the theorem.
Proposition 29.10 The Sullivan model (AV ®AZ, d) can be chosen so that
Q(Z) = 0, ? = ? ?? and
p>0
? ?
(i) d: Zp —> 1®(?*?)?_?? ? A>^m-^V ® Zp-q \®A>m+^m-^V, ? > 0.
L,=o J
(it) The quotient differential, d, in AZ satisfies
d: Zp A (A2Z) nkerd, ? > 1, n > 1 .
(Hi) The inclusion of (AV ® (it ? Zo),d) in (AV <g> AZ, d) is a quasi-isomorphism
(Here (AZ), is the grading induced by the grading Zt.)
proof of Theorem 29.9: Regard (AV ®(lk®Zo),d) as a (AV, d)-bimodule
by writing
U?AV®(]k®Z0) .
Then define a product in AV ® (Ik© Zo) by setting
A simple calculation shows that this product is associative and makes
(AV <g> (Jfc ? Z0),d) into a commutative cochain algebra. We denote this cochain
algebra by (A,d). It is immediate that ? : (AV, d) —> (A,d) is a morphism of
cochain algebras as is the restriction 77^ : (A, d) —> (AV, d) of ?.
We prove the theorem by constructing a morphism ? : (AV ® AZ, d) —> (A, d)
of cochain algebras such that ?? = X. Then ?' = ?)?? '¦ (AV® AZ, d) —> (AV, d)
is the desired retraction.
Rational LS-Category 395
First observe (Proposition 29.10 (iii)) that ? restricts to a surjective quasi-
isomorphism (AV ®(k© ZQ),d) —> (AV/A>mV,d). Thus its kernel, /,. satisfies
? (I, d) = 0. Moreover, since ?(?0) = 0,1 = A>mV ? (AV ® Zo).
Now we construct ? inductively to satisfy
(? + ??.??) : Zp—> I , p>0.
First, since Zo c I we may set ?? = ?, ? ? Zo. Suppose ? is constructed in
AV ® ? (?<? ? Z<n), and simplify notation by writing W = Z<p @Z<n. Set
U = (A2IF)P_! ? (?+V ® Wp) ? (A^mV ® W<p) ? A>mV .
Then Proposition 29.10 (i) asserts that dZp C U. On the other hand, a trivial
calculation (using the fact that ? preserves products and restricts to the identity
in AV) shows that (? + ????)? C /. Thus for ? G Zp , (? + ????)?? is a cocycle
in /.
Since H(I) — 0 there is a linear map h : ?™ —> I such that (? + ????)?? =
dhz. Extend ? to an algebra morphism ? : AV ® A(\V ? Zp) —> ? by setting
?? = hz !p[T}Ahz, ? G Zp. The equations
and ????? = ——????? ,z G Z"
p+ 1 ?
p+
are immediate from the definitions and together give ??? = ???, ? ? Zp. Finally
? + ???? = ? ·' ? ? —> I. This completes the inductive step, and the proof of
the theorem. ?
Corollary cat(AV, d) < m if and only if there is a diagram of (AV,d)-module
morphisms
(AV,d) (P,d) (AV,d)
(M,d)
in which ?? = id, 7 is ? quasi-isomorphism and A>mV · M = 0.
proof: The definition of cat(AV, d) < m provides such a diagram. Conversely
given such a diagram we may (in the notation of Theorem 29.9) factor 70 as
(AV,d) A (AV/A>mV,d) A (M,d). Then, because (AV ® AZ,d) is semifree,
?? lifts through the morphism 7 to yield a morphism of (AV, d)-modules ? :
(AV ® AZ,d) —> (P,d) such that ?? ~ ?? (as morphisms of (AV,^-modules).
Then ??\ ~ ??? = ? ? = ??. Since 7 is a quasi-isomorphism, ?? ~ a (Proposi-
tion 6.4(ii)).
396 29 LS category of Sullivan algebras
Set ? = ?? : (AV <g> AZ, d) —> (AV, d). Then ?? = ??? ~ ?? = »dAy. Now
iiG?A)[l] = [1] and so ???A) = 1. Since 7?? is AV-linear. 7?? = id,\V. By
Theorem 29.9 cat(AV, d) < m. D
Example 1 Field extension preserves category.
Let (AV,d) be a minimal Sullivan algebra with V = {V*} ·>9? and let ? be a
field extension of k. Then (AV, d) <g> ? is a minimal Sullivan aTgebra over K. We
show now that
cat(AV, d) = cat ((AV, d) <g> K) .
Suppose cat ((AV, d) <8> K) = m and let
(AV, d) <g> ? 2 ^ (p, rf) E. - (?V, d) <g> ?
(M, d)
be morphisms of (AV, d) <8> K. modules as in the Corollary to Theorem 29.9. In
particular, A>mV ¦ M = 0 and ?? = id. Let ? : k —> ? the inclusion and let
? : ? —> k be a it-linear map such that ?? = id. Then the identity of (AV, d)
factors as
(AV, d) -24 (P, d) -^ (AV, d) ,
and 7 is also a quasi-isomorphism of (AV, d)-modules. Thus the Corollary to
Theorem 29.9 asserts that cat(AV, d) < m.
Conversely, if cat(AV, d) = m we can apply — <8> ? to the diagram of the
Corollary to conclude cat ((AV, d) <g> K) < m. D
Example 2 Rational category of smooth manifolds.
Let M be a simply connected smooth manifold with rational cohomology of
finite type, and let (AW, d) —y Aor(M) be a minimal Sullivan model for the
cochain algebra of C°° differential forms on M. We observe that
cato M = cat(AT-F,d) .
In fact, as we saw in §11, Adr(M) is connected by quasi-isomorphisms of
commutative cochain algebras to Api(M; Q) <g> M. Thus if (AV. d) is a rational
minimal Sullivan model for M then (AW, d) = (AV. d) <8> M. It follows now from
Example 1 (and Proposition 29.4) that cat0 M = cato(AV, d) = caX(AW,d). D
(f) The model of (AV,d) —» (AV/A>mV,d).
Recall that Proposition 29.10 describes the structure of a Sullivan model
? : (AV®AZ, d) ^> (AV/A>m V, d) of the surjection ?, where (AV, d) is a minimal
Sullivan algebra and V = {V1} .>o. Here we provide the proof, beginning with
two preliminary steps.
Rational LS-Category 397
Step 1 The quadratic part of the differential.
Let d\, be the quadratic part of the differential in AV. Construct a (AV, de-
module, (AV® (k®M),di) by requiring that d\ : Mn —» A>mV ? (A+V <g>
M<n) and that
a = #(^) : ?" ? ? (A>mV ? (A+V ® M<n)) .
Since F = {Vl}.>o any element of degree ? in A>mV ? (A+V <g> M) has the
form ? = ? + y + ~z with ? G Mn, y G A+V <g> M<n~l and ? G A>mV. If ?? = 0
then ? is in the kernel of the map a above and so ? = 0. Then d(x + y) = 0
and so by construction, ? + y is a coboundary in A>mF ? (AF <8> AiT<n). It
follows that ? (A>mF ? (AV <8> M), di) = 0. In particular, a quasi-isomorphism
of (AV, d\ )-modules
?: (AV®(Jfc®M),di) A (AV/A>mV,d1)
is defined by C(l) = 1 and ?(?) = 0.
Bigrade AV by putting (AV)P'* = APV. Then di is homogeneous of bide-
gree A,0). The inductive procedure above defines a bigradation in M : M =
? Mp'* so that d\ is homogeneous of bidegree A,0) with respect to the in-
p>m
duced bigradation in AV <g> (Jk ? ?), denoted by [AV <g> (jfe ? M)]p'q. We call ?
the filter degree.
The key observation is that ?>??·* = 0; i.e.,
Lemma 29.11 M = Mm·*.
proof: As in the Example in §14(b) extend (AV, di) to a minimal relative
Sullivan algebra (AV <g> AV, ?>)_with H(AV <g> AV, D) = Jk. As shown in that
Example, D : V —> A+V <8> AV, and the linear part of D is an isomorphism
V -^ V of degree 1.
Filter (AV <g> AV, D) by the ideals A-PV <g> AV. In the resulting spectral
sequence the differential in Eq is zero and so the isi-term is a Sullivan algebra
(A V<8>A V, ?) extending (A V, d\ ). As with D the linear part of ? is an isomorphism
V A V. It follows that (AV®AV, ?) is contractible and hence H(AV®AV, ?) =
L· Note that ? is homogeneous of bidegree A,0) with respect to the bigradation
(AV <g> AV)p·* = ApV <g> AV.
Next recall (Proposition 6.7) that if (?,?) is any semifree (AV, di)-module
then —<8>avN preserves quasi-isomorphisms. Thus we obtain quasi-isomorphisms
(AV/A>mV <g> AV, ?) A [AV <g> (Jfe ? ?)] <8>?? [?V <g> AV] —> (k ? ?, 0) .
It follows that k ? ? and H (AV/A>mV ® AV,5) are isomorphic as bigraded
spaces.
Finally, use the short exact sequence
0 —> (A>mV® AV,o) —> (AVtg)AV,J) —> (AV/A>mV®AV,5) —> 0
398 29 LS category of Sullivan algebras
to obtain an isomorphism H+ (AV/A>mV <g> ??,?) A H (A>mV ®AV,o) of
bidegree A,0). The source is concentrated in bidegrees (p, *) with ? <m and the
target in bidegrees (q, *) with q > m. It follows that H+ (AV/A>mV ® AV, ?)
is concentrated in bidegrees (m, *). D
Step 2 A (AV,d)-semifree resolution for (AV/A>mV,d).
The differential d in AV satisfies: (d - dx) : (AV)*·· —» (AV)^+2'*, since
(AV)P·* is just ApV. We shall now extend (AV,d) to a (AV, d)-module
(AV ® (it ? M),d) such that
d-dx: [AV ® (Jfe ? M)]Pt' —» [AV ® (Jfe ? M)]^p+2'* .
For this it is sufficient to suppose d constructed in M<n and to extend it to Mn.
Observe first that any d-cocycle ? of degree n + 2 in [AV ® (Jfe © jif<n)]-m+3'* is
the d-coboundary of an element in [AV ® (Jfe ? M<n)]-m+2>*. In fact, write ? =
n+2
? Xii where x; has filter degree r, some r > m+ 3. Then dz = 0 implies d\xr —
i=r
0. Since H (AV ® (Jk ? ?), di) is concentrated in filter degrees < m it follows
that xr = ???' with x' of filter degree r—1. In particular, x' G (A-2V ® ?)"+1?
n+2
AV c AV®(Jfc©M<n). Now z—dx' = ? Vi witn 2/? of filter degree i. Continue
i=r+l
in this way to obtain ? = du with u G [AV (8) (Jfe ? M<n)]-m+2'*.
We now extend d to M" as follows. If tu G M" then diu; G AV ® (Jk ?
M<n), because V = {Vl}.>o. Thus ddity is a d-cocycle of degree ? + 2 in
[AV®'(Jfe©M<n)]-m+3f·, because M = M··. This implies ddxw = du for
some u G [AV <g> (Jk ? M<n)]-m+2'* as we observed just above. Extend d by
setting dw = d\w — u as w runs through a basis of Mn.
This completes the construction of (AV ® (Jk ? M), d). Since d(M) C A>mV©
(AV ® M) a morphism
C : (AV ® (Jfe ? M),d) —» (AV/A>mV, d)
of (AV,d)-modules is defined by ? = g and ?(M) = 0. (Thus if we forget
AV
differentials ? coincides with the morphism ? of Step 1). In particular filtering
by [AV ® (Jk ? ?)]-?·* and by A^pV/(A>mV). we obtain from ? a morphism of
spectral sequences which at the J?i-term is just
??(?) = ? : (AV ® (Jfe ? M),dx) -> (AV/A>mV,d!) .
Since ? is a quasi-isomorphism so is ?: ? is ? (AV, d) semifree resolution of
(AV/A>mV,d).
Finally, the same argument as used in the observation at the start of the
construction of d gives
Rational LS-Category 399
Lemma 29.12 If w ? [AV <g> (k ? M)]>r'" is a d-cocycle and if r > m then
w = du for some u G [AV <8> (k ? M)]-r'*. D
We now turn to the
proof of Proposition 29.10: Set Zo = A/, so that the (AV, d)-module of
Step 2 is written (AV <g> (k © Zo),d). Extend d and the quasi-isomorphism ? of
Step 2 (uniquely) to a morphism C : (AV <8> AZ0,d) —» (AV/A>m V, d) of cochain
algebras.
Next suppose by induction that this is extended to a morphism
C·. (AV®A(Z<p(BZ<n),d) -> (AV/A>mV,d)
of cochain algebras such that ? (?<? ? Z<p) = 0 and conditions (i) and (ii) of
the Proposition -are satisfied. Simplify notation by writing W = Z<p ? Z<"
and extend the quotient Sullivan algebra (AW, d) to a quadratic Sullivan algebra
{A(W ? Z?),d) by requiring that d : Z? A (AHV)^ A herd.
Let z G Z?. Then ddz = ? + y with
? G 0 A>9(m-1)V ® (?2^)^^! and y G ??^+^'?? <g> Wp-q-i .
q<P q<P
The component of d(x + y) in AV <8> A3H^ is just (id ? d)x. Since d(x + y) — 0
so is (id <g> d)x = 0. By (ii) of the Proposition, ? = (id <8> d)x' for some x' G
0A>9(m-l)y0Iy .
P
It follows that ? = d(dz—x') is a cocycle in AV®(k@W). Thus its component
in AV <8> IV is an (id® d)-cocycle. By (ii) of the Proposition this component is
in AV <8> Zq. Thus a careful inspection shows that
? G (?>1+?(??)?® ?? ©A>m+1+p(m~1)V .
Apply Lemma 29.12 to conclude that ? = <2? with ? G (A>p(m-^V <8> Zo) @
i\>m+p{m-\)y Extend d by setting dz = d? - ?' — ? as ? runs through a basis
of Zp. Extend ? by setting ? {Zp) = 0. This completes the inductive step and
hence the construction of (AV <8> AZ, d) and of ?.
To check that ? is a quasi-isomorphism it is sufficient to establish (iii): the
inclusion of (AV <g> (k ? Zo), d) in (AV <g> AZ, d) is a quasi-isomorphism, because
the restriction of ? to (AV <g> (it ? Zo), d) is a quasi-isomorphism (Step 2).
Filter both sides by the submodules A-p V®— to obtain a morphism of spectral
sequences with ?O-term the inclusion (AV 0 (k ? Zo), 0) —> (AV <g> AZ, id<g>d).
It is thus sufficient to show that k ? Zo ^ (AZ, d). But in view of (ii) this is
precisely the assertion in Example 7 of §12(d). D
(g) The Milnor-Moore spectral sequence and Ginsburg's theorem.
400 29 LS category of Sullivan algebras
Let (AV, d) be a minimal Sullivan algebra with V = {V*} . Filtering by the
ideals Fp = A-PV yields a spectral sequence, the Milnor-Moore spectral sequence
for (AV, d). This spectral sequence was introduced in §23(b) where it was shown
that
(Eo,do) = (AV,0)
and
where L is the homotopy Lie algebra of (AV, d). Moreover Eqo is just the asso-
ciated bigraded algebra of H(AV,d):
Eg _ FpH(AV)/Fp+lH(AV) ,
where FPH(AV) is the image of H(A^pV,d) in H(AV,d).
On the other hand, e(AV, d) is the largest integer ? such that the image of
H(A~rV,d) in H(AV,d) is non-zero. We restate this as
Proposition 29.13 The Toomer invariant e(AV, d) is the largest integer r such
that E^m(AV,d) ? 0. In particular,
??* (AV, d) = 0 p> cat(AV, d) .
Finally we establish the Sullivan algebra version of a theorem of Ginsburg [64],
for which a simple proof was later given by Ganea [62].
Theorem 29.14 Suppose (AV, d) is a minimal Sullivan algebra and V =
{Vl}i>0. If cat(AV,d) = m then the Milnor-Moore spectral sequence collapses
at some Ee, ?<m + l; i.e. Em+i = Eoo·
proof: Recall the (AV,d)-semifree resolutions
C(Jfe) = (AV ? (Jfe ? M(Jfe)), d) ^> (AV/A>kV, d)
constructed in Step 2 of §29(f). We denote AV <& (k @ M(k)) simply by Q(k).
Recall that Q(k) is bigraded with AW = (AV)P'* and M(k) = M(k)k-* (Lemma
29.11). Moreover, it follows from the construction that d : Q(k)P'* —» <2(a;)^p+1'*.
Our first step is to construct a commutative diagram of (AV, c?)-modules of
the form
(Q(k + m),d) —i— AV/A>m+kV
(Q(m),d)
Rational LS-Category
401
such that ? : Q(k + m)p'* —> Q(m)-P'* for all ? and ? is the identity on AV.
(Here ?A) = 1 !) For this it is sufficient to construct ? in M(k + ??) and we
suppose by induction this is done in M(k + m)<n.
Let ? G M(k +m)n. Then ?? G [AV <g> (Jk ? ?/(A: + m)<n)]>fc+m'*. Thus ^dz
is defined and ??? G Q(m)>k+m'*. By Lemma 29.12 we may write ??? — au
with u G <2(m)-fc+m"\ Extend ? by setting y?z = u.
This completes the construction of ?. Since ? and ? preserve nitrations
?? (M(k + m)) = 0 = ?? (M(k + m)) and thus the diagram commutes.
Now suppose caX(AV,a) < m. Then there is a morphism ? : (Q(m),a) —>
(AV, ?) of (AV, d)-modules extending the identity on (AV, ?). In particular,
? : Q(m)p·* —> A>p~mF. It follows that ?(?)?? vanishes in M(k + m) and thus
the diagram
Q(m + k) ? Q(m)
gC(m+k)
AV
commutes.
Finally let z G ??*'*, some i > m. Then 2 is represented by an element
w G A-fcy such that aw 6 A-*+fy. To show ?(? = 0 it is sufficient to find a
cocycle w' G A-kV such that ty - w' G A>Ay.
But since aw ? A>k+mV, ?(?? + k)w is a cocycle in AV/A>k+mV. Write
?(?? + k)w — C(m + k)u for some cocycle u G Q(m + k). Then g(k)w = ??(?? +
k)w = ??(?? + k)u = ?(^????. Thus the cocycle w' = ???, has the desired
properties, since g(k)w' = g(k)w.
This shows ?( - 0, ? > m + 1 and so ??? = 2?m+i. ?
(h) The invariants meat and e for (AV, d)-modules.
Fix a minimal Sullivan algebra (AV,a). This topic relies heavily on §6, often
without explicit reference. In particular morphism means morphism of (AV, ?)-
module and homotopy is the relation defined at the start of §6(a). For any
(AV, d)-module, (?, ?), we may then make the following constructions:
• a semifree resolution (?, ?) -^ (?, ?).
• the surjections g(k) : (P, a) —» (P/A>kV -?,?).
• homotopy commutative diagrams of (AV, c?)-module morphisms,
(P(k),d)
(P,d)
(P/A>kV-P,a) ,
where c(k) is a semifree resolution of (P/A>kV *P,d).
402 29 LS category of Sullivan algebras
These constructions are unique 'up to quasi-isomorphism'. Indeed, if (Q, d) -=*
(M, d) is a second (AV, d)-semifree resolution then there is a quasi-isomorphism
a : (Q, d) -=» (P, d) of (AV, d)-modules. Moreover
id®Ava : AV/A>kV®AV Q —» AK/A^V ®Av ?
is also a quasi-isomorphism, and this is just the quotient map a(k) : Q/A>kV · Q
—» P/A>kV-P. Thus a(fc) lifts to a quasi-isomorphism ?(k) : (Q(k),d) —»
(P(&), d) such that Q(k)?(k) ~ a(A;)C(A;). Automatically then ?(*)? ~ ?(k)\(k).
In particular, the following definition is independent of the choice of semifree
resolution and constructions above:
Definition (i) The module category of (M,d), mcat(M, d), is the least integer
m for which there is a morphism ? : (?(m), d) —> P(d) such that ?\(??) ~ id.
(ii) The Toomer invariant of (M,d), e(M,d), is the least integer r
such that H(g(r)) is injective.
Proposition 29.15 For a minimal Sullivan algebra (AV,d) and any (AV,d)-
module, (M,d):
(i) e(M,d) < mcat(M,d).
(ii) mcat(M,d) < mcat(AV,d).
(Hi) If V = {^*}i>2 ???? mcat(AF,d) = cat(A7,d).
proof: (i) This is immediate from the definitions.
(ii) Suppose mcat(AV, d) = k and construct a homotopy commutative
diagram
(AV,d) * ~ (N,d)
(AV/A>kV,d)
and a morphism ? : (N,d) —> (AV, d), such that ? is a semifree resolution and
?\ ~ id. Let (P, d) be a semifree resolution for (M,d), and apply — ®AV ? to
this diagram. This yields
(P,d) ^ (N®AVP,d) ?- (P,d)
Q
(P/A>kV-P,d)
with 77'?' ~ id. It follows that mcat(M, d) < k.
Rational LS-Category 403
(iii) Let C : (AV(g>AZ,d) —> (AV/A>mV,d) be a minimal Sullivan
model for the surjection ?. It is in particular a (AV, d)-semifree resolution. If
cat(AV,d) = m there is a morphism ? : (AV <g> AZ,d) —» (AV,d) of Sullivan
algebras restricting to the identity in (AV,d). In particular, ? is AV-linear and
so mcat(AV, d) < m.
Conversely, if mcat(AV, d) = m then there is a morphism ? : (AV<g> ??, d) —>
(AV, d) of (AV, d)-modules such that ?? ~ id, X denoting the obvious inclusion.
But this implies ?(?\) = id and so 7??A) = 1. Since ?? is AV-linear, ?? = id.
Now Hess' theorem 29.9.asserts that cat(AV, d) < m. D
Finally, suppose (M,d) is a (AV,d)-module. The dual (AV,d)-module,
Hom(M, L·), of J;-linear functions is defined by
df = -(-l)desffod and (?·/)(?) = (-1)??6?
for / G Hom(M, Jt), ? G AV and ? G M.
Theorem 29.16 [55] Let (AV, d) be a minimal Sullivan algebra such that V —
{} and has finite type. Then
cat(AV, d) = e (Hom(AV, Jfe), d) .
Lemma 29.17 If a quasi-isomorphism a : (Q,d) —> (N,d) of (AV, d) -modules
factors as (Q,d) —> (iV',d) —> (iV,d), un?/i A>rV-iV' = 0, i/ien
mcat(Q, d) < r .
proof: We lose no generality in assuring (Q,d) seniifree. It follows from our
hypotheses that a factors as (Q,d) -^4 (Q/A>rV-Q,d) A (N,d). This yields
a homotopy commutative diagram
(Q,d)
in which Q(r) is a semifree resolution. Since ??? ~ ?g(r) = a and 'q is a
quasi-isomorphism it follows that 7?? ~ id. Thus mcat(Q,d) <r. ?
proof of Theorem 29.16: Denote (Hom(AV,J:), d) by (M,d). The finite
type restriction implies that (AV, d) = (Hom(M, Jk),d). Since cat(AV, d) =
mcat(AV, d) > e(M, d) — Proposition 29.15 — we have only to show that
e(M, d) > mcat(AV, d) .
404 29 LS category of Sullivan algebras
Let ? : (P,d) -^ (M,d) be a semifree resolution. Since (Hom(M,]k),d) =
(AV, d) this dualizes to a semifree resolution Hom(</?, k) : (AV, d) -=» Hom(P, k).
Let ? = Hom(y?,A)l G Hom(P, jfc).
Now suppose e(M,d) = r. Then the surjection ? : (P,d) —» (P/A>rV -P,d)
is injective in homology. It follows that the dual, Hom(p, A), induces a surjection
? (Hom (P/\>rV · P, A)) H· H (Hom(P, Jfe)) .
In particular there is a cocycle / G Hom (P/A>TV · P, k) such that [f ? ?] = [?].
The quasi-isomorphism AV ^» Hom(P, It) is given by ? ?—> ?·?. Thus it
factors as
(AV,d) A (Hom (P/A>rV-P,k),d) Hom<>e'*\ (Eom(P,k),d)
where ?? = ? · /. Now for any g G Hom (P/A>rF · P, k), x G P/A>rV ¦ ? and
? GA>rFwehave(*-p)(x) = ±g($-x) =0. Thus A>rF- Hom (P/A>rV-P,k
= 0 and Lemma 29.17 implies that mcat(AV, d) < r. D
Corollary Let X be a simply connected topological space with rational homol-
ogy of finite type, and rational minimal Sullivan algebra (AV,d). Then
proof: Apply Proposition 29.4.
Rational LS-Category 405
Exercises
1. Suppose that the homogeneous space M = SpE)/SUE) admits a minimal
model of the form (A(a,b,x,y,z),d) with deg ? = 6, deg b — 10, deg ? = 11,
deg y = 15, deg ? = 19, da = db — 0, dx = a2, dy = ab and dz = b2. Compute
2. Let F be a coformal space and X = ??? en. Supposing catF — ? > 1. Prove
that catX < catF.
3. Using 24.5, 24.7 and 29.5, prove that any sub Lie algebra of a free graded Lie
algebra is free.
4. Let X — (CP2 V S2). Denote by ?, ? and 7 generators, respectively, of
7T5(CP2), 7T2(S2) and vr2(CP2). Consider the spaces Y = X U[a>0] e7 and ? =
CP2 ? S3. Using minimal models prove that the map / : CP2 V53 -> F which
restricts to the identity on CP2 and represents the class [?, y] when restricted
to S3, extends, once localized, to a continuous map / : Zq -» Yq such that ?»/
is injective. Prove that catoF = 3. Deduce that there is no mapping theorem
for the invariants eo and for the rational cup length.
30 Rational LS category of products and fibra-
tions
In this section the ground ring is an arbitrary field h of characteristic zero.
Lusternik-Schnirelmann category is not well-behaved on products and fibra-
tions. For example, a simple argument shows that for topological spaces Y and
max(cat Y, cat Z) < cat(F xZ)< cat Y + cat ? ,
and inequalities are sharp: for each one there is a non-trivial example where
the inequality is an equality. For general fibrations ? : X —> Y with fibre F,
moreover, the best that can be asserted is
0 < cat A" < (cat Y + l)(cat F + 1) - 1 .
This section analyzes the behaviour of the rational category of products and
fibrations. For products cat0 behaves well: we establish the recent result with
Lemaire [55]: Y and ? are simply connected with rational homology of finite type
then
cato(F ? Z) = cato Y + cat0 ? .
(The case ? = Sn had been established earlier by Hess [90] and Jessup [98].)
In the case of fibrations of simply connected spaces with rational homology of
finite type, the inequalities above remain sharp if cat is replaced by cato- With
reasonable assumptions, however, they can be improved. For example, if F is an
odd sphere then
cato A' < cato Y + 1 ,
while if cato F < oo then
cato A' > cato F - dim d, (?????(^) <8> Q) ,
<9» ® Q : vr,(F) ® Q —> n.-i(F) <8> Q denoting the connecting homomorphism.
Finally, we strengthen the Mapping theorems 28.6 and 29.5 for the special case
of a fibre inclusion.
This section is organized into the following topics:
(a) Rational LS category of products.
(b) Rational LS category of fibrations.
(c) The mapping theorem for a fibre inclusion.
(a) Rational LS category of products.
We begin with the classical
Proposition 30.1 If Y and ? are normal topological spaces then
max(cat K, cat Z) < cat(F ? ?) < cat Y + cat ? .
Rational LS-Category 407
proof: The first inequality follows from the fact that Y and ? are retracts
of ? ? ? (Lemma 27.1). For the second, suppose cat Y = m and cat ? = ?.
Then Y and ? are respectively retreats of an m-cone P' and an ?-cone Q'
(Theorem 27.10). We may write ?' ~ ? = {p0} C Pi C - - - C Pm with Pk+l =
Pk Ufk CAk, and Q' ~ Q = {q0} C Qi C · - - C Qn with Qe+l = Qe Ugt CBt. It
is clearly sufficient to prove cat(P x Q) < m + ?.
Set(PxQ)r = U PkxQe. Then (PxQ)r-(P xQ)r-i is the disjoint union
k+t=.r
of the contractible open subsets (Pk - Pk-i) x (Qr-k ~ Qr-k-i) of (? ? Q)r;
thus it is contractible in (? ? Q)r- Let pk 6 Pk — Pk-i and qe ? Qe — Qe-i be
?
the vertices of the cones CAk and CBt and set U = (? ? Q)r — [J (pk,qr-k)·
k=0
Since (? ? Q)r = ((? ? Q)r - (? ? Q)r_i) U U it follows that cat(P ? Q)r <
catU + 1. On the other hand, U deformation retracts onto (? ? Q)r-\ with
deformation ? : U x I —> U given explicitly as follows: If (p, q) 6 (? ? Q)r-i
then H(p,q,t) = (p,q). If ? = (?, s) 6 CAk and q = (b,s') 6 CBr-k then
s + s' > 0 and we set
Thus t/ ~ (PxE)r_! and cat U = cat(PxQ)r_!. Now we havecat(PxQ)r <
cat(P ? Q)r-i + 1 and hence cat(P ? Q) <n + m. D
The following classical example shows that the inequality of Proposition 30.1
can be strict. Fix a prime ? and let Mn{p) = Sn U/n CSn where /„ : 5" —> 5"
induces multiplication by ? in homology. Clearly we may take fn+\ to be the
suspension ?/? of/„ and so Mn+1(p) ^ ???(?). In particular, catMn(p) = 1,
n> 2.
Now if c is a second, different prime the inclusion i : Mm(p) V Mn(q) —>
Mm(p) ? Mn(q) induces a homology isomorphism (easy calculation). Thus the
Whitehead-Serre theorem 8.6 asserts that i is a weak homotopy equivalence.
But these spaces are CW complexes and so i is a homotopy equivalence (Corol-
lary 1.7). Thus
cat(Mm(p) ? Mn{q)) = cat (Mm(p) V Mn(q)) = 1 .
Recently, Iwase [95] has constructed a 2-cell complex Y such that
cat(K x 5") = cat Y = 2 ,
thereby providing a considerably more dramatic example. Rationally, however,
we have
408 30 Rational LS category of products and fibrations
Theorem 30.2 [55]
(i) If Y and ? are simply connected topological spaces with rational homology
of finite type then
cato(F ? Z) = cato Y + cat0 ? .
(ii) If (AV,d) and (AW,d) are minimal Sullivan algebras with V = {Vz\
and W — {W%} i>2 9r<ided vector spaces of finite type then
cat ((AV, d) ® (AW, d)) = cat(AV, d) + cat(AW, d) .
proof: The category of the rational Sullivan minimal models for ?, ? and
? ? ? is just the rational LS category of ?, ? and ? ? ? (Proposition 29.4).
Moreover the models for Y and ? satisfy the conditions of (ii) (Proposition 12.2)
and their tensor product is the model for F ? ? (Example 2, §12(a)). Thus (i)
follows from (ii) when Hz = Q.
For (ii) we first let (M,d) and (N, d) be respectively any (AV, d)-module and
any (??47, d)-module and notice that (M, d) ® (TV, d) is a (AV, d) ® (AW, d) in the
obvious way: (? ® ?) -(m ® n) = (-l)dee* deem<? . m ® ? · ?. We show that
e ((M, d) ® (N, d)) = e(M, d) + e(N, d) ; C0.3)
the theorem then follows from Theorem 29.16 which asserts that cat(—) =
e(Hom(—,&)) for Sullivan algebras satisfying the hypotheses above.
It remain to prove C0.3). We may suppose (Ai, d) and (N,d) are respec-
tively (AV, d)- and (AW, d)-semifree; in this case the tensor product is obvi-
ously (AV, d) ® (AW, d)-semifree. If e(M, d) = m and e(N, d) = ? then the
surjection ? : (M,d) ® (N,d) —> (M/A>mV-M,d) ® (N/A>nW · N, d) is in-
jective in cohomology. Since kex-? D A>m+n(V ? TV)-(M ® N) it follows
that e((M,d) ® (N,d)) < m + n. On the other hand, if u 6 A^mV-M and
? e A>nW · ? are cocycles representing non-trivial cohomology classes in H(M)
and H(N) then u ® ? 6 A^m+n(V ? TV) -(M ® N) represents a non-trivial co-
homology class, so e ((M, d) ® (iV, d)) > m + n. D
(b) Rational LS category of fibrations.
In this topic we consider both topological spaces and Sullivan algebras. Thus
? ? ? C0.4)
will always denote a fibration with fibre F in which ?, ? and F are simply
connected. Similarly,
(AV, d) —> (AV ® AW, d) C0.5)
will always denote a minimal relative Sullivan algebra in which (AV, d) is itself
a minimal Sullivan algebra and V and W are concentrated in degrees > 2. We
Rational LS-Category 409
recall from Theorem 15.3 that if k = Q and C0.5) is a Sullivan model for C0.4)
then the Sullivan fibre (AW, d) is a Sullivan model for F and that the connecting
homomorphism d* <8> Q : vr,(F) <g> Q —>¦ ?*_!(^) ® Q is dual up to sign to the
linear part of the differential do : W —> V (Proposition 15.13). Finally, in this
case Proposition 29.4 asserts that cat(AF, d) = cat0 Y, cat(AV®AW, d) = cat0 X
and cat (AW, d) = cat0 F. These results and notation will be used without further
reference throughout this topic.
Example 1 The relative Sullivan algebra (A(x, y), dy = xr+1) —> (A(x, y, u, ?),
dy = xr+1,dv = un+1 -x).
In this example we take ? and u to be cocycles respectively of degrees 2(n +1)
and 2. The quasi- isomorphism ? (?, y) —> (Ax/xr+1,d) shows that H (A(x,y),d)
is a commutative model for (A(x,y),d). Thus (Corollary to Proposition 29.3)
cat(A(x,y),d) = nil (Ax/xr+1) = r. Similarly (A(u,v),d) -^> Au/un+l and
(A(x,y,u,v),d) -^ (Au/u(r+1)(n+1),0). Thus
cat(A(x,y,u,v),d) = (n + l)(r + l) - 1
= (cat (A(x, y), d) + 1) (cat (A(u, ?), d) + l) - 1 .
Proposition 30.6 The fibration ? : X —> Y C0.4) satisfies
catX < (caty + l)(catF+l) -1
and this inequality is best possible, even for rational spaces.
proof: Let cat F = m so that Y is the union of m + 1 open sets Ua each
contractible in A'. The inclusion \a of p~1(Ua) in A" is then homotopic to a map
P~l{Ua) —> F M- A' and it follows that catAa < catj < cat F (Lemma 27.1).
Thus ?~?(???) is the union of (n + 1) open sets each contractible in A', and so
catX + 1 < (catF + l)(catF + l).
To see that this inequality can be sharp recall from Proposition 17.9 that
spatial realization | | converts a relative Sullivan algebra to a Serre fibration.
Thus in Example 1, \A(x, y, z, u, v),d\ —> \A(x,y),d\ is a Serre fibration with
fibre |?(??, ?), d\. Denote \A(x, y), d\ by Y and convert this to a fibration A" —> Y
with A" ~ \A(x,y,u,v),d\ and fibre F ~ \A(u,v),d\. Now by the Corollary to
Proposition 29.4 the LS category of the realization is the category of the model.
Thus Example 1 translates to
= (r + l)(n + l)-l = (catY + l)(catF + 1) - 1 . ?
Under reasonable hypotheses it is possible to improve considerably on Propo-
sition 30.6 for rational LS category. First we have
410 30 Rational LS category of products and fibrations
Proposition 30.7
(?) If dim W is finite and W is concentrated in odd degrees then
cat(AV ® AW, d) < cat(AV, d) + dim W = cat(AV, d) + cat(AW, d) .
(ii) If ir*(F) ® Q is finite dimensional and concentrated in odd degrees and if
Y has rational homology of finite type then
cato X < cato Y + dim ?,(F) ® Q = cat0 Y + cat0 F .
proof: (i) The inequality is just the Mapping theorem 29.5(ii) applied to the
inclusion (AV, d) —> (AV ® AW, d). The equality dim W = cat(AW, d) is Exam-
ple 6, §29(b).
(ii) is an immediate translation of (i). ?
Proposition 30.8 (Jessup [98])
(i) IfH(AV®AW,d) ^% H(AW,d) is surjective then
cat(AV ® AW, d) > cat(AV, d) + nil H (AW, d) .
(ii) If H*(X;Q) —> H*(F;Q) is surjective and if Y and F have rational ho-
mology of finite type then
cato X > cat0 Y + c0F .
proof: Put H = H(AW, d) and choose a linear map ? : (?, 0) —> (AV®AW, d)
such that ?(?)?(?) = id. Then ? extends to a morphism id·? : (AV,d) ®
(H,0) —> (AV®AW,d)of (AV, d)-modules. It preserves the filiations (AV)^p®
? and (AV)-P ® AW and the resulting morphism of spectral sequences is an
isomorphism of .&>-terms. Thus id · ? is a quasi-isomorphism. It follows that
there is also a quasi-isomorphism ? : (AV ® AW, d) —> (AV ® H, d) of (AV, d)-
modules because (AV ® AW, d) is also (AV, d)-semifree.
Suppose nil ? (AW, d) = r and let ? 6 ? be the product of r cohomology
classes in H+. Each of these is the image of a cohomology class in H+(AV ®
AW, d). The product of representing cocycles is thus a cocycle ? 6 AV ® AW
such that ?? — 1 ® ? 6 A+V ® H<deguJ. Now define AV-linear maps
a : (AV,d) —> (AV ® AW, d) and (id® /) : (AV,d) ® ? —> (AV,d)
by setting ?(?) = ? ·? and requiring /(u>) = 1- Then (id® ^?? = 1 and hence
(id ® f) ? ? ? ? = ic
Rational LS-Category
411
On the other hand, write AV <g> AW = AZ, with ? = V ? W. Since ? is the
product of r cocycles of positive degree, ? 6 A-rZ. Thus for any m, ? induces
a morphism ? : (AV/A>mV,d) —> (AZ/A>m+rZ,d). This leads to the diagram
(AV, d)
\v
(Pv,d)
(AZ,d)
(Pz,d)
in which ?? and ?? are respectively (AV, d)- and (AZ, d)-semifree resolutions
and ??, ?? and /? are respectively 'homotopy lifts' of ??, Qz and ???· Thus
????? ~ CzAzu: as (AF, d)-linear maps; since ?? is a quasi-isomorphism, /3?^ ~
?^? (Proposition 6.4(i)).
Finally, observe that since [?] maps to the non zero cohomology class ?, [?] ? 0
and cat(AZ, d) > e(AZ, d) > r. Suppose cat(AZ, d) = m + r. Then there is a
morphism ? : (Pz,d) —> (AZ,d) of (AZ, d)-modules such that ??? = id. Set
? = (id ® ?)??? : (Pv,d) —> (AV, d). Then in the diagram
(AV,d)
(AV/A>mV,d)
we have ??? = (id® /)????? ~ (id® /)????& = (id® /)?? = id\v- Thus
the Corollary to Theorem 29.9 asserts that cat(AV, d) < m, i.e. cat(AZ, d) >
cat(AV,d)+n\\H(AWj).
This establishes (i), and (ii) is an immediate translation. D
(c) The mapping theorem for a fibre inclusion.
In the case of a fibre inclusion the Mapping theorems 28.6 and 29.5 can be
strengthened as follows.
412 30 Rational LS category of products and fibrations
Proposition 30.9
(i) Ifca,t(AW,d) < oo then d0 = 0 in Weven. Conversely, if do = 0 in Weven,
then
dim Im d0 < cat(A W, d) < cat(AF <g> AW, d) + dim Im d0 .
(ii) Suppose Y and F have rational homology of finite type. If cat0 F < oo
then 9. ®Q = 0 in ????(?) <g>Q. Conversely, if d* ®Q = 0 m 7rodd(r) ®Q,
dim Im(9* <g> Q) < cat0 -F < dim Im(9* <g> <Q>) + cat0 X .
proof: Divide (AV®AW, d) by A-'2V®W to obtain a quotient cochain algebra
((k <8> V) <8> AW,<5). If {ui} is a basis of F then E = id <8> d + ??* <g> oi and the
#i are derivations of (AW, d). Thus if ni = deg#* then ?? restricts to a Gottlieb
element fi : Wni —> k. Now suppose dou>i = Vi, 1 < i < r. Then fi(wi) = 1
and fj(wi) = Q, j ? i- Thus Proposition 29.8 (ii) asserts that if cat (AW, d) < co
then each ?, is odd and r < cat(AW,J). It follows that d0 = 0 in Weven
and dim Im do < catiAW, d). The second inequality of (i) is just the Mapping
theorem 29.5(ii).
(ii) is an immediate translation. D
Example 1 Fibrations with catX = 0.
If cat X = 0 then X is contractible. Modify the constant map PY —> pt —> Y
to a fibre preserving map PY —> X, which is (automatically) a homotopy
equivalence and so induces a weak homotopy equivalence flY —> F. This map
is then a rational homotopy equivalence and so cat0 ?.? = cat0 F.
Thus we have two possibilities (Example 3, §28(d)): either cat0 F = oo or else
?,(?7) <g>Q is finite dimensional and concentrated in odd degrees. In the latter
case, 7r„(F) <8> Q is finite dimensional and concentrated in even degrees; thus Y
has a Sullivan model of the form (AFeven,0). In particular, cato(F) = oo:
cat X = 0 ==$¦ max (cato Y, cat0 F) = oo . D
Example 2 Fibrations with cat X = 1.
We construct examples of fibrations with catX = cato X — 1, and
cato ^ = ? and ? < cato F < ? + 1 ,
for any ? > 1. For this, let pt : Sj —> Sf be a copy of the Hopf fibration, with
? ? ?
fibre Sf, 1 < i < n. Convert the composite map V Sj —> ? 5/ —> ? ^ into
i=l i=l i=l
a fibration ? : X —> Y with fibre F : Y = f[ S* and ? ~ V 5Z7. In particular
cat X — cato X = 1, and cat0 Y = cat ? = ?.
Rational LS-Category 413
Now ?. E*) ® Q = Qea ® Qe'a with degea = 4 and deg< = 7 (Example 1,
§15(d)) and e'a is in the image of ?,(??) <g> Q by construction. Thus each e'a G
Im ?* (?) <g> Q. On the other hand ?' is 6-connected and so <9* <8> Q is a injective
in tt4(F) <g> Q. It follows that ker(<9* <g> Q) = ?7(?) <g> Q and Proposition 30.9(ii)
asserts that ? < cat0 F < ? + 1. D
When the fibration ? : X —> Y has a cross-section we obtain a lower bound
for cato X:
Proposition 30.10 // the fibration ? : X —> Y has cross-section then
cato X > max (cat0 Y, cat0 F) = cato(F V F).
proof: Recall from the Example in §28(a) that max (cato Y, cato F) = cato(Y V
F). Let s : Y —> X be the cross-section: ps = ???. Thus exhibits F as a retract
of X so cato Y < cato X and it exhibits vr*(p) as surjective, so the inclusion
j : F —> Y induces an injection 7r*(j) ® (Q> of rational homotopy groups. By the
Mapping theorem 28.6, cato F < cat0 X as well. D
The next example shows that Proposition 30.10 is best possible.
Example 3 Fibrations with cato X = max (cato Y, cato F).
For any simply connected topological spaces Y and ? convert the map
(idy, const.) : Y V ? —> Y into a fibration ? : X —> Y with A'~KV Z,
as described in §2(c). The inclusion Y —> ? \? ? then defines a cross-section s
of this fibration; in particular the fibre F is simply connected.
Moreover, the inclusion ? —> ? ? ? defines an inclusion i : ? —> F. Let
j -. F —> X be the inclusion. Since the fibration has a cross-section, ?» (j) <8> Q
is injective. Since ji is homotopic to the inclusion ? —> Y V ?, ?» (ji) ® Q is
injective. Hence ?» (i) <g>Q is also injective and the Mapping theorem 28.6 asserts
that
cato ? < cato F < cat0 X -
Thus cato X = max (cato Y, cato Z) = max (cato Y, cato F). D
Exercises
1. Let F ? X A F be a fibration in which ??, F and F are simply connected.
Prove that cat A' < (cat i + 1) ¦ (cat ? + 1) — 1.
2. Let X be a simply connected space and let ? be a prime number. Consider
the Moore space Mn(p) = Sn Up en+1. Prove that if X is a rational space, the
inclusion X V Mn(p) -> ? ? ??(?) is a homotopy equivalence. Supposing that
tf+(X;Q) ^ 0. Prove that cat(X ? ??{?)) ? cat(X) + cat(Mn(p)).
3. Let F ? ? ->· F be a fibration in which A', F and F are simply con-
nected. Suppose that dimH>n(F;Q) = 0, and that there exists an element
? g Hn(X;Q) such that ??(?)(?) f 0. Prove that cato(I) > cato(F) +1.
414 30 Rational LS category of products and fibration
4. Let / : X ->· Y be a continuous map between simply connected CW complexe
of finite type. Suppose that K2n(f) ® Q is injective for ? > 2. Prove that
cato/ < catoX < cato/ + dim (ker7rodd(/)
31 The homotopy Lie algebra and the holonomy
representation
In this section the ground ring is an arbitrary field h of characteristic zero.
Recall from §21 (d) that the homotopy Lie algebra, Lx, of a simply connected
topological space X is the graded vector space ?,(??) <8> k, equipped with
a Lie bracket defined via the Whitehead product in ?,(?). In particular, (cf.
Example 2, §21) each ? 6 Lx determines the linear transformation adz : Lx —>
Lx, given by
ad ? : y >—> [?, y] , y 6 Lx .
In this section (Theorem 31.17) we show that
• //cato X < co then for each non-zero ? 6 (Lx)even of sufficiently large de-
gree there is some y 6 Lx such that the iterated Lie brackets
[x, [x, [x,... [x, y]... ]]] are all non-zero.
A linear transformation ? : V —> V in a graded vector space is called locally
nilpotent if for each ? 6 V there is an integer n(v) such that ??^? = 0, and
an element ? in a graded Lie algebra is called an Engel element if adz is locally
nilpotent. Thus we may restate the result above as: z/cato X < co then the non-
zero Engel elements in (Lx)even are concentrated in finitely many degrees. In
particular, if cato X < co and if (dim Lx)even is infinite then Lx is not abelian.
We shall use this to show cato X — co in an example in which eo(X) = 2 and
the Milnor-Moore spectral sequence for X collapses at the Ez-term.
The theorem above follows as a special case of a theorem of Jessup [99], which
will be our principal objective in this section. For this we consider a Serre
fibration
p:X —> Y
with fibre F at a basepoint yo 6 Y, and such that Y is simply connected with
rational hoinology of finite type. Associated with this fibration is a right repre-
sentation (§23(c)) of the homotopy Lie algebra LY in H*(F;jk), the holonomy
representation for this fibration.
This representation, which is an important invariant of the fibration, is con-
structed as follows from the space ? ?? ?? of §2(c). First, the right ac-
tion of UY on X xY PY makes H* (X xY PY;Jk) into a right ?*(??;??-
module as described in §8(a). Second, there is a natural weak homotopy equiv-
alence j : F —> ? ?? ?? (Proposition 2.5) and we use the isomorphism
H*(j;fc) *? identify H9(F;3c) as a right H,(ilY;Je)-modu\e. Finally, since
Hm(QY;Jk) = ULY (Milnor-Moore Theorem 21.5) the action of H*(QY;k) in
Hr(F; Jk) restricts to a right representation of Ly.
Definition This representation of Ly in H, (F; Ik) is the holonomy represen-
tation for the fibration ? : X —> Y.
416 31 The homotopy Lie algebra and the holonomy representation
Observe now that any ? 6 Ly determines two linear transformations:
ad ? : LY —> LY and hi ? : H*(F; k) —> #.(F; A) ,
where hi ? is simply the restriction of the holonomy representation to x. Jes-
sup's theorem asserts that if X and F are also simply connected with rational
homology of finite type then:
• // ?, (?) ® Jk is surjective and if there are r linearly independent elements
a?? 6 (-i'y)even such that adz* and hlxt· are both locally nilpotent, then
cato X > cato F + r .
Jessup's theorem is proved by a careful analysis of the holonomy representation
in terms of the Sullivan model of the fibration ? : X —> Y. For this we first
introduce the holonomy representation for an arbitrary minimal relative Sullivan
algebra
(AV, d) —> (AV ® AW, d)
in which V = {V*}i>9 and W = {^'}.>9 are graded vector spaces of finite type
and (AV, d) is itself a minimal Sullivan algebra.
Recall that the homotopy Lie algebra L of (AV, d) is the graded Lie algebra
defined by
V = Hom(sL, Jfc) and (AV, di ) = C* (L) ,
where d\ is the quadratic part of the differential d in AV (§21(e) and Example 1,
§23(a)). Now filter (AV ® AW, d) by the ideals F? = A^PV ® AW to obtain a
first quadrant spectral sequence (??,??). Its So-term is just
(??,??) = (AV ® AW, id ® d) ,
d denoting the quotient differential in AW, and so the .??-term, (?\,?\), is a
(AV, di)-module of the form
(??, ??) = (AV®H(AW,d),Si)
in which <5i : H(AW,d) —* V ® H(AW,d). Thus (cf. B3.6)) a right representa-
tion of the homotopy Lie algebra L in H(AW,d) is defined by
Definition This representation of L in H (AW, d) is the holonomy representa-
tion for the relative Sullivan algebra (AV, d) —>· (AV ® AW, d).
Now suppose the relative Sullivan algebra (AV ® AW, d) is a model for the
fibration ? : X —>· Y, as in A5.4). This determines a quasi-isomorphism
(AW,d) -=> APL(F), and so identifies H(AW,d) = H*(F;k). On the other
hand, Theorem 21.6 identifies L with Ly , so that the holonomy representation
for the fibration is a right representation of L in H*(F; k). In Theorem 31.3 we
show that
Rational LS-Category 417
• The holonomy representations of L in H(AW,d) and in H*(F;k) are dual
up to sign.
This permits the translation of hypotheses on the holonomy representation of
Ly in H*(F;jk) into conditions on the differential in the Sullivan model (AV ®
AW,d) where they can be applied, in particular, to prove Jessup's theorem.
An important aspect of the holonomy representation for any relative Sullivan
algebra (AV, d) —> (AV ® AW, d) is that it can be expressed in terms of deriva-
tions of the quotient Sullivan algebra (AW, d), (a derivation ? in a differential
graded algebra (A,d^) is a derivation in A such that 6dA = (—l)degedA6). More
precisely, if Vi is a basis of V, derivations #* of (AW, d) are defined by
d(\ ® ?) - 1 ® ?? - Y^ vi ® ??? 6 A.-2V ® AW , ? 6 AW .
i
Thus by definition the spectral sequence differential ?? : ? (AW, S) —> V <8>
H(AW,d) is given by <5?[?] = ? ?, ® ?(??)[?]. It follows that if xj is the dual
i
basis of L ((vi; sxj) = 1 or 0 as i = j or i ? j), then
a-Xi = (-l)deeXi{deea+1)H(ei)a , ? 6 H(AW,d) . C1.1)
Formula C1.1) expresses the holonomy representation for the relative Sullivan
algebra in terms of the derivations ?(??). However, the derivations ?% themselves
carry more information. For example, if V = fov and deg ? is odd then the entire
differential in Av <g> AW is given by
dv = 0 , and d(l <g> ?) = 1 <g> ?? + ? <g> ?? , ? 6 ??47 .
In particular, any derivation <?' of (AW, d) with deg ?' = 1 — deg ? determines a
relative Sullivan algebra Av —> (Av <8> AW, d') by the formula d! = l®d + v®6'.
Note as well that (in general) the holonomy representation is characterized by
¦ (AV®H(AW,d),S1) = C* (L;H(AW,d))
(cf. §23(c)). In particular, the spectral sequence above converges from
E2 = ExtUL (Je, H (AW, d)) =*· H(AV ® AW, d) .
This section is organized into the following topics
(a) The holonomy representation for a Sullivan model.
(b) Local nilpotence and local conilpotence.
(c) Jessup's theorem.
(d) Proof of Jessup's theorem.
(e) Examples.
418 31 The homotopy Lie algebra and the holonomy representation
(f) Iterated Lie brackets.
(a) The holonomy representation for a Sullivan model.
Consider a Serre fibration ? : X —> Y with fibre inclusion j : F —> X,
and such that F, X and Y are simply connected and have rational homology of
finite type. Let ? : (AV, d) —> (AV <8> AW, d) be a minimal relative Sullivan
algebra that is a minimal Sullivan model for the fibration in the sense of A5.4);
in particular we have the commutative diagram
APL(Y)
(AV,d)
Apl(p)
Apl(X)
Apl(F)
C1.2)
(AV ® AW, d)
(AW, d) ,
in which ??? and m are minimal Sullivan models and m is a Sullivan model.
Use H(m) to identify H(AW,d) = H*(F;]k) = Horn (//.(F; *),*), and use
Theorem 21.5 to identify the homotopy Lie algebra Ly for Y with the homotopy
Lie algebra L for (AV, d). Recall the two holonomy representations of L, one in
H(AW,d) and one in H^(F;k), defined in the introduction to this section.
Theorem 31.3 These two representations are dual (up to sign):
proof: As in §16(b) we may apply the construction in §15(a) to the path space
fibration q : PY —> Y to obtain a commutative diagram
APL(Y)
APL(PY)
APL(QY)
(AV, d)
(AV ®AV,d)
- (AV,0)
of Sullivan models. Now consider the pullback diagram of iiF-fibrations
X xY PY * PY
f
X = Y ¦
Rational LS-Category 419
Diagram C1.2) provides a Sullivan model m : (AV ® AW, d) -=> APL(X) and
a Sullivan representative for ?, ? : (AV, d) —> (AV ® AW,d), such that mA =
???,{?)???- We may therefore, as in §15(a), construct
t = APL(f)m-ApL(g)n:(AV®AW,d)®Kv(h.V®AV,d)-*ApL(XxYPY).
Moreover, Proposition 15.8 asserts that ? is a Sullivan model of the form
?: (AV ® AW ® AV, d) -=> i4PL(X x
(Note that AV ® AW and AV ® AV are sub cochain algebras.)
Next consider the composite Serre fibration ? ? / ·. ? ? ? ?? —y Y. The fibre
at yo is F x CIY and the inclusion of the fibre is the continuous map
? : F ? CIY —» ? ?? ?? , ? : (?, ?) ·—+ U'^) ·7 , ? e F, y e ?? .
A straightforward check shows that in this case diagram A5.4) has the form
APL(Y) Ap^°f) APL(X ????) ^^ APL(F ? ??)
¦n C1.4)
(AV,d) (AV ® AW ® AV, d) — (AW,d) ® (AV,0).
Let ii be a cocycle in (AW,d) representing ? 6 H*(F;Jc). If ? : AW —>
V ® AW is the map defined by ?? - 1 ® ?? - ?? 6 A^2V ® AW, then by
definition ?(?) = ?? and
a-z = (-l)desu+des*(tf@)a;5:r) . C1.5)
On the other hand, the weak homotopy equivalence j : F —> ? ? ? ?? is
represented by the surjective quasi-isomorphism ? · id ·? : (AVigiAW^AV, d) —>
(AW, d). Let w G AV <g> AW ® AV be a cocycle that maps to u. Then it follows
from diagram C1.4) that for ? G H*(F;lk),
(?,?-?) = {H*(a)H*(j)-la,?®hur(x))
= (H(e-id)[w],?®hur(x)) .
But by construction we may write
w = 1 ® u ® 1 + w\ ® 1 + y^l ® Uj ® Vj + ?
where W! G V®AW, m G AW, Vi G V and ? G A^2(Ve V)-A(V® AW® AV).
From dw = 0 we deduce that dui = 0 and hence that
(?,?.?) = (_l)Wegz T([Ui},?)([Vi},hur(x)) .
420 31 The homotopy Lie algebra and the holonomy representation
ex
Furthermore, if d0 : V -^> V is the linear part of the differential in AV <g> AV we
also deduce from dw = 0 that Qu + (id®d)wi + ?(~l)degUi desVid0Vi <g>Uf = 0.
i
Thus C1.5) becomes
(a-x,?) = -
Finally, recall that L = Ly = ?*(?.?) <g> k and that sLy is identified with
?*(?) <8> k via sz = — (— l)de&xd~1x, where 9, is the connecting homomor-
phism of the path space fibration (§21(e)). Thus the formula in the proof of
Proposition 15.13 becomes (d0Vi;sx) = (v^x) = ([vi], hur(x)), where (?,?·?) =
d3d '
Again suppose ? : X —> Y is a Serre fibration with fibre F, and with A', Y and
F simply connected and ha\ring rational homology of finite type. The holonomy
representation of the homotopy Lie algebra Ly in the homology H*(F; h) of the
fibre is an important measure of the non-trh'iality of the fibration: the holonomy
representation for the product fibration is trh'ial. More generally we ha\'e
Proposition 31.6 Let ? : X —> Y be a Serre fibration with fibre F, and sup-
pose Y is simply connected with rational homology of finite type. IfH*(X; k) —>
H*{F]k) is surjective then the holonomy representation of Ly in H,:(F;Ji) is
trivial.
proof: Let (AV <g> AW, d) be a minimal Sullivan model for the fibration as
in A5.4). Then for any cohomology class ? G H (AW, d) there is a d-cocycle
? G AV <g> AW of the form ? = 1 <g> ?? + *? ? such that ?; G AiV <g> AW
and ?? is a- <i-cocycle representing a.
As in the introduction, choose a basis V{ of V and define derivations ?{ of
(AW, d) by d(l <g>?) - 1 ® ?? - ??{ ®?{? G A^2V <g> AW. Then (formula C1.1))
the holonomy representation in H(AW, d) is given by ? · Xi = ±?(??)?, where ??
is the dual basis of the homotopy Lie algebra L of (AV, d). Here we have dty = 0
and so ??{ <g> ^?? = -(id <8> rf)*i. Thus each ?(?{) = 0 and the holonomy
representation in H*(F;Jk) is trivial (Theorem 31.3). D
(b) Local nilpotence and local conilpotence.
Recall that a linear map 7 : M —> M in a graded vector space is locally
nilpotent if e\'ery ? G M is in the kernel of some power 7fc of 7. We shall make
frequent use of the dual notion:
Lemma 31.7 Suppose ? : M —> M is a linear map of non-zero degree in a
graded vector space M = {Mp} eZ of finite type. Then the following conditions
are equivalent:
(i) The dual linear map in Hom(M, k) is locally nilpotent.
Rational LS-Category 421
(ii) For each ? there is an integer k(p) such that Mp ? lmok^ = 0.
(in) If (wk)k>o is an infinite sequence of elements of M such that aWk+i = Wk,
k>0 then wk = 0, k > 0.
oo
(iv) ? Im ?* = 0.
k=0
Definition A linear transformation of non-zero degree in a graded \rector
space of finite type is called locally conilpotent if it satisfies the conditions of
Lemma 31.7.
proof of Lemma 31.7: (i) ·?=?- (ii): Denote the linear map dual to ? by /.
Then
(M, fk (Hom(M, Jfc)p)> = (Mp ? Im ?*, Hom(M, k)p) .
Thus if (ii) holds then fk^ = 0 in Hom(M, Jk)p, pGZ, and / is locally nilpotent.
Conversely, if (i) holds then /fc(p) = 0 in Hom(A/, fo)p for some k(p), because
this vector space is finite dimensional. Thus Mp ? Imak^ = 0 and (ii) holds.
(ii) <=>¦ (Hi): Clearly (ii) => (iii). If (ii) fails then for some ? and
each jfe, Mp ? lmak ? 0. Set Ak = (?^1) (?" - {0}). Then Ao ?- ?? ?-
A2 ?— · · · is an infinite sequence of linear maps between non-void affine spaces.
For dimension reasons the sequences .4^ D ?(.4^+1) D ?2 (Ak+2) D ··· must
stabilize at some integer K(k) : ?? (Ak+n) = ??^ (Ak+K^) for ? > K(k).
Put Ek = ??^ (Ak+K(k))· Then for ? large enough
a(Ek+1) =?(?? (Ak+l+n)) = ??+1 (Ak+n+i) = Ek -
Thus we may inductively construct elements Wk G Ek so that aWk+i = Wk-
Moreover, since Ak = (crk+l) (Mp — {0}) no element in Ak is zero, so u>k ? 0,
Jfe>0.
(ii) ^=^ (iv): Clearly (ii) =?· (iv) and the reverse implication follows
from decreasing sequence Mp D Mp ? Im ? D Mp ? Im ?2 D ¦ ¦ ¦ and the fact
that Mp is finite dimensional. ?
Example 1 The holonomy representation.
Suppose (AV, d) —> (AV <g> AW, ci) is a relative Sullivan algebra as in the
introduction to this section and let (vi) be a basis of V. Let L be the homotopy
Lie algebra of (AV, d) and denote by hi' ?, ? G L, the holonomy representation
of L in H(AW,d). Recall from the introduction that derivations ?-? in (AV, d)
axe determined by the equations
d(l ® ?) - ( 1 ® ?? + ? Vi ® ??? J G A^2V <g> AW , ? G AW .
Formula C1.1) states that HFi) coincides (up to sign) with hl'xi, where (??) is
the basis of L dual to the basis (v{). Thus Lemma 31.7 asserts that the folknving
conditions are equivalent:
422 31 The homotopy Lie algebra and the holonomy representation
• hi' Xi is locally conilpotent.
• For each ? there is an integer k(jp) such that Hp(AW, d) Dim ?@i)h^ — 0.
If (ak)k>o is an infinite sequence of elements of H(AW,d) such that
H{9i)oik+l = ak, k > 0, then ak = 0, k > 0.
D
Example 2 The adjoint representation.
Let L be the homotopy Lie algebra of a minimal Sullivan algebra (AV, d) in
which V = V-2 is a graded \-ector space of finite type. Each ? G L determines the
linear function V —> k given by ? \—>¦ (v;sx). Extend this to a derivation, ??,
in AV and define a derivation ?? in (AV, d) by setting ?? = ??? — (-1)?^???? d.
Because (AV, d) is minimal ?? preserves A+V. Define fx : V —> V by requiring
fx is the linear part of ??. We show now that
(fxv;sy) = (-l)d^xde^(v;s[x,y}) , ? G V, y G L .
This formula exhibits fx as the dual (up to sign) of ad x. In particular, fx is
locally conilpotent if and only if ad ? is locally nilpotent.
For the proof of C1.8) note that fx = ???? - (-l)desVxnxdi, where dx is the
quadratic part of d. Then use §21(e) to compute
(fxv;sy) = -(-l)de^^xd1v;sy) = (-l)d
We shall need one more observation about local conilpotence: its good be-
haviour with respect to spectral sequences. Indeed, suppose ? : (M, d) —> (M, d)
is a linear transformation of a graded vector space of finite type and suppose fur-
ther that both ? and d preserve a filtration of M of the form
M = F°M DFlAID---D FPM D ¦ ¦ ¦
in which f]FpM = 0. This determines a cohomology spectral sequence (Ei,d{)
?
and a morphism ??(?) : (Ei,di) —> (Ei,di).
Lemma 31.8 If some Ei(a) is locally conilpotent then so is ?(?).
proof: If ?(?) is not locally conilpotent then there is an infinite sequence of
non-zero classes ak G H (M), such that H(a)ak+i = ak, k > 0. Let p(k) be
Rational LS-Category 423
the greatest integer such that ak has a representing cocycle in Fp(fc)il/. Since
p(k) > p(k + 1) > · · · there is a p and a ko such that p(k) = p, k > ko.
Let Zk be the affine space of cocycles in Fp representing ak, k > ko. Then
?? (Zk+n) 3 cn+1 (Z/j+n+i) D · · · is a decreasing sequence of finite dimensional
affine subspaces and hence Ak = f] ?? (Zk+n) is non void subspace of Zk- Exactly
?
as in Lemma 31.7 it follows that a(Ak+i) = Ak, and so there is a sequence of
cocycles zk G Ak such that ? Zk+i = Zk and Zk represents a^.
Since ? = p{k) and Zk G Fp, Zk represents a non-zero class [zk] G Ef'*. But
clearly Ei(a)[zk+i] = [zk], k > ko, and so if ? (?) is not locally conilpotent then
Ei(a) is not locally conilpotent either. D
Examples 1 and 2 allow us to prove a technical lemma that is important for
the proof of Jessup's theorem, and in §33. Consider a minimal Sullivan algebra
of the form (Av ® AU ® AW, D) in which
• ? has odd degree and Dv = 0.
• (Av ® AU, d) —> (Av ® AU ® AW, D) is a relative Sullivan algebra.
Then a derivation, ?, of the quotient. Sullivan algebra (??7 <g> AW, d) is defined
by D(l <g> ?) = 1 ® ~?? + ? <g> ??.
Next, let L be the homotopy Lie algebra of (Av <g> AU,D) and denote by ad
and by hi' the adjoint representation of L, and the holonomy representation of
L in H(AW,d), where d is the quotient differential in AW. Define ? G L by
(?; sx) = 1 and (U; sx) — 0. Then we have
Lemma 31.9 With the notation and hypotheses above suppose ad ? is locally
nilpotent and hi' ? is locally conilpotent. Then ?(?) is locally conilpotent.
proof: First observe that ? preserves AU and also A+U, because (Av®AU, D)
is a minimal Sullivan algebra. Next, let ? be the derivation in Av ® AU defined
by ??? = (w;sx), w G jkv ? U; thus ?? = 1 and rj(U) = 0, and thus setting
? = ?·? - (-1)?^^?? we have
?A <g> ?) = 1 <g> ?? , ? G AU .
Define / : U —>· U by the condition ?-f-.U—i- A^2U. Then Example 2 states
that / is dual (up to sign) to ad x. In particular / is locally conilpotent.
Next, filter AU® AW by the ideals Fp - A^PU®AW. Since ? preserves A+U
it presents this filtration and in the corresponding spectral sequence we have
?? (?) =ef® id + id ®?(??) ¦ AU ® H (AW, d)^AU® H(AW, d) ,
where #/ is the derivation in AU extending /. Now ?(??) is locally conilpotent
by hypothesis, and we have just observed that so is /, because ad ? is locally
nilpotent. Given a positive integer n choose ? so that for k > 0:
U^n ? fk (U^N) = 0 and H^n(AW, d) ? HFv)k (H^N(AW, d)) = 0 .
424 31 The homotopy Lie algebra and the holonomy representation
Then the formula above for E\ (?) implies that
[APU ® H(AW, d)] nDE1 @)* [.\PU ® H(AW, d)] ^ip+1)N = ? , jfe > 0 .
Hence ?\(?) is locally conilpotent and thus so is ?(?), by Lemma 31.8. D
(c) Jessup's theorem.
Consider a fibration
V.X-+Y
of simply connected topological spaces with simply connected fibre F and such
that Y and F (and hence X) have rational homology of finite type. Let <9, <g> Jc :
?*(?) ® He —> tt»-i(F) ® Ik denote the connecting homomorphism and let hi
denote the holonomy representation of the homotopy Lie algebra Ly in H* (F; ]k).
Theorem 31.10 (Jessup [99]) Suppose in the fibration ? : X —> Y that d, ®
k = 0. Assume there are r linearly independent elements ? ? (Ly)even such that
both ad ? and hi ? are locally nilpotent. Then
cato X > cato F + r .
We shall deduce Theorem 31.10 from its translation into Sullivan algebras.
For this we consider a minimal relative Sullivan algebra
(AV, d) —»· (AV ® AW, d)
in which V = V-2 and W = W-2 are graded vector spaces of finite type, and
(AV, d) is itself a minimal Sullivan algebra. Choose a basis v\, v2, ¦ ¦ - of V such
that degu; < degUj+i and define derivations O,· in the quotient Sullivan algebra
(AW, d) by requiring
d(l ® ?) - 1 ® ?? - ? Vi ® ??? e A^2V ® AW , ? € AW .
i
Then let (x{) be the dual basis of the homotopy Lie algebra L of (AV,d).
Theorem 31.11 Suppose in the relative Sullivan algebra (AV, d) —> (AV ®
AW, d) that the linear part of the differential in AV ® AW is zero. Assume that
in the basis of L there are r elements Xi of even degree such that ad ?? is locally
nilpotent and ?(?{) is locally conilpotent. Then
cat(AK® AW, d) > cat(AW, d) + r .
We show first that Theorem 31.11 does imply Theorem 31.10. Indeed, choose
(AV, d) —> (AV ® AW, d) to be a rational Sullh'an model for the fibration ? :
Rational LS-Category 425
X —> Y as described in A5.4). Then (AV, d) is a minimal Sullh'an model for
Y and L = Ly. Thus the linear part of the differential in AV ® AW is zero,
because it is dual to <9, <g> k (Proposition 15.13). Suppose there are r linearly
independent elements ? G Leven such that ad ? and hi ? are locally nilpotent.
Choose a basis Vi,v%,... ?? V such that degux < degu2 < · · · and so that these
r elements appear as a subset ???,...,??? of the dual basis (x{) oiL. Then adz*,,
is locally nilpotent. Moreover, H(9iu) is dual (up to sign) to hlzit,, by formula
C1.1) and Theorem 31.3. Thus H(9it/) is locally conilpotent.
This sho\vs that the minimal Sullivan model of ? : X —> Y satisfies the
hypotheses of Theorem 31.11. Now let (AU1, d) and (AW1, d) be minimal rational
Sullivan models for X and for F. Then (AU',d) ® k and (AW',d) ® k are
minimal Sullh'an models (over k) for X and F. It follows that cat(AV<g>Aiy, d) =
cat(AC/', d) = cato X and cat(AtV, d) = cat(AW', d) = cat0 F (Example 1, §29(e)
and Proposition 29.4). Thus Theorem 31.10 follows from Theorem 31.11.
The proof of Theorem 31.11 will occupy the next two topics. First, in §31(d),
we shall consider the special case that V = kv and degu is odd, so that AV is
the exterior algebra Av. Then in §31 (e) we shall use induction and the mapping
theorem to prove the theorem in general.
(d) Proof of Jessup's theorem.
Consider first a minimal Sullh'an algebra of the form (Av <g> AW, d) in which
deg ? is odd. Thus the differential is described by: dv = 0 and
d(l ® ?) = 1 ® ?? + ? ® ?? , ? G AW , C1.12)
where ? is a derivation in the quotient Sullh'an algebra (AW,d).
In this case Theorem 31.11 reduces to
Proposition 31.13 If ?(?) is locally conilpotent then
cat (Au ® AW, d) > cat (AW, d) + 1 .
proof: Letcat(Au®AW,d) = m + l. The ideals ?® (AW,d) and v®(A>mW,d)
are in particular (Av®AW, d)-modules. Let ? : (Q, d) -=> v® (AW/A>mW, d) be
a (Av ® AW, d)-semifree resolution for the quotient module. Define lq G H(Q)
by ?(?)??> = [?® 1].
Next, observe that Q extends to the (Av®AW, d)-semifree module (Q®N,d)
denned as follows: iV is a graded space with basis (an)o<n<oo and degan =
n(degv — 1), the module action is ? -(? ® an) = ?? ® an for ? G Av ® AW,
? G Q, and the differential is gh'en by
d(z ® ao) = dz ® oq and d(z ® an) = dz® an+vz® ??-? , ? > 1
We identify Q with Q ® ao via ? <—> ? ® ao-
426 31 The homotopy Lie algebra and the holonomy representation
The proof of the proposition then consists of the following four steps.
Step 1: Construction of a morphism of (Av ® AW, d)-modules 7 : (Q, d) —>
(Av ® AW, d) such that H(j)lq = [v ® 1].
Step 2: Extension of 7 to a morphism ? : (Q ® N,d) —> (Av ® AW, d).
Step 3: Construction of a homotopy from ? to a morphism ? with image in
v®AW.
Step 4: Proof that caX(AW, d) <m.
We now carry out this program.
Step 1: Construction of a morphism of (?? ® AW, d)-modules 7 : (Q,d) —>
(Av ® AW, d) such that H(j)lq = [v ® 1].
Let I(m + 1) = A>m+1 (v®W) <zAv® AW and let ? : (?, d) ^> (Av ® AW/
I(m + \),d) be a (Av ® AW,d)-semifree resolution. Define iP e H(P,d) by:
H(C)ip = [1]. Since cat(Au ® AW, d) = m + 1 there is a morphism 77 : (P, d) —>
(Av ® AW, d) of (Av ® AW, d)-modules such that ?(??) = [1]. (This is just the
definition of cat(Au <g> AW, d) if we take for (P, d) the minimal Sullivan model of
the surjection Av <g> AW —> Av <8> APF/7(m + 1)-)
Next observe that the inclusion of the ideal v®(AW, d) in (Av®AW, d) factors
to define a morphism
? : ? ® (AW/A>mW, d) —»· (At; ® AW/I(m +\),d)
of (Av ® AW,d)-modules. Lift ? to a morphism ?' : (Q,d) —> (P,d) such that
??' ~ ^.
Finally set j = ??' : (Q,d) —»· (At; ® AW,d). Since ?(?)?(?')?? =
?(?)?(?)??> = ?(?)[?® 1] = [?] · 1 = ?^@^ = #(?([?]·*?) we have
H(tp')iQ = [?] ¦ Lp. Thus H(j)lq = [v® 1],
Step 2: Extension of 7 to a : (Q ® N,d) ^ (?? ® AW, d).
Extend f to 6v : (Q®N, d) —> v® (AW/A>mW, d) by setting ??(?>®??) = 0,
? > 1. In the diagram
(Q ® N, d) ? ® (AW/A>mW, S)
we may construct the morphism r so that ?? ~ ^ (Proposition 6.4(i)). Moreover
it is immediate from the proof of this proposition that we may suppose r restricts
to the identity in Q, because ?/v and ? coincide in Q. Thus
? = ? ? ?' ? ? : (Q ® ?, d) —> (?? ® AW, d)
Rational LS-Category 427
restricts to 7 in (Q,d).
Step 3: Construction of a homotopy from a to a morphism ? with image in
? ® AW.
It is in this Step that we apply the hypothesis that ?(?) is locally conilpotent.
More precisely, we establish
Lemma 31.14 Suppose (xn, zn)n>o is an infinite sequence of pairs of elements
xn,zn 6 AW satisfying:
dzn = 0 and ???+? = zn + dxn , ? > 0 .
Then there is an infinite sequence of elements yn ? AW such that
dyn = zn and 9yn+i = yn + xn , ? > 0 .
proof: Observe first that each [zn] G f| Im HF)k = 0, since [zn] = ?(?)[??+1] =
k
?(?J[??+2] = ¦ ¦ ¦ ¦ Thus the affine spaces An = d~l(zn) are non-void and finite
dimensional. Affine maps
—> An+i —> An —> > Ao
are defined by an(y) = 6y — xn , y G An+\. For each ? this gives the infinite
decreasing sequence of affine spaces
An DlmanD Im(an ? ??+?) D · · · D Im(an ? · · · ? an+k) D · · · ·
For dimension reasons this sequence must stabilize at some k(n) : Im(an ? · · · ?
cxn+p) =Im(ano.--an+fc(n))forp > k(n). Put En = Im(ano· · -oan+fe(n)). Then
an(En+i) = En since, for ? sufficiently large, an(En+i) = an(Iman+i ? ··· ?
ctn+p) = En- Thus we may choose an infinite sequence of elements yn G En such
that an+iyn+i = yn,n> 0. Thus 0(yn+i) = yn + Xn- Since En C An = d~l(zn)
we also have dyn = zn, ? > 0. ?
We now complete Step 3 by constructing a homotopy ? from ? to a morphism
? satisfying the stronger condition
?(Q®ao)Cv®AW and ?(Q®an)=0, n>\. C1.15)
For this, write Q = Av <g> APF <g> M, where M decomposes as the direct sum
00
? = ? Mfc such that d(M0) = 0 and d(Mk+i) C Av <g> ??-?7 <g> M<fc. Denote
fc=0
and Av <g> APF ® M<fc respectively by Qt and Q<t·
Now any linear map g^ : Mk®N —> Av®AW extends uniquely to a Av®AW-
linear map Gk ¦ Q <8> ? —>· ?? ® ??-?7 such that Gk{Mi ® N) = 0, ? # A;. A
428 31 The homotopy Lie algebra and the holonomy representation
sequence of linear maps, gk : Mk ® ? —> ?? ® AW, determines in this way the
oo
Av <g> A W-linear map G = ? Gk ¦ Q ® ? —> ??® \W.
fc=0
Suppose now by induction that gi, i < k of degree —1 are constructed so that
a' = a - [ ? dGi + Gid] satisfies condition C1.15) in Q<k ® N. We shall
\i<k J
construct g/, so that a- ^ dGi +Gid I satisfies C0.15) in Q<k ® N. If (gk)
\i<k )
f °°
is the infinite sequence constructed in this way then ? = a — ^ dGi + Gi
\i=0
f
satisfies C1.15) because ? restricts to ? — [? dGi + Gid I in Q<k ® N.
Vi=o /
It remains to construct gk, given a'. Define ?? ® AM^-linear maps fn,qn :
Q<k —> AW by the equations
?'(?®??) = /?? + ?(????) , ?>0.
The condition da = ad then implies
dfn = fnd and #/n+i - fn = dqn+i + qn+id , n>0.
Recall now that we suppose a' = 0 in Q<fc<8>an, ? > 1 and that a' (Q<k ? ao) C
? ® AW. Since d : Mk —> Q<k it follows from this that
fn°d : Mk —> 0 , ? > 0 and qn ? d : Mk —> 0 , ? > 1 .
Thus if ? G Mk the equations above reduce to
dfnZ = 0 , ? > 0 and ?/?+?? — fnz = dqn+iz , ? > 0 .
We may thus apply Lemma 31.14 with zn = fnz and xn = qn+iz to find a
sequence of elements yn € AW such that
dyn = Zn and 0yn+i = yn + ?? , ? > 0 .
Now define ^ : Mfc ® ? —> AW by setting gk(z ® an) = yn where (yn) is a
sequence as above for z, and ? runs through a basis of Mk- Then d<7fc(z <g> an) =
/„? and 9gk(z ® an+1) = 5fcB <g> an) + ???+?2, ? > 0, ? G Mk- Moreo\'er
[a' - (dGk + Gkd)} (z®an) = fnz+v(qnz)-dgk(z®an)-v9gk(z®an)+vgk(z®an--l)
where ?_? =0. Thus the right hand side is zero for ? > 1 and reduces to
v(qkZ — 9gk(z ® oo)) if ? = 0. Since a' and ? coincide in Q<k ® ?, the inducth'e
step is complete.
Step 4: Proof that cat(AJ?, d) < m.
Rational LS-Category 429
Define a (?W,d)-module structure in (Q ® N,d) by setting
$-(z®an) = JT(-l)P( P + U )(???)?®??+?, ?????, zGQ .
p=0 \ ? /
(Since ? decreases degrees this series is a finite sum). By Step 3, ? : (Q®N, d) —>
v®(AW, d) is a morphism of (Av®AW, d)-modules, vanishing on Q®an, ? > 1.
It follows that ? is a morphism of (AW, <i)-modules.
Next, let zq be a cocycle in Q representing the class lq of Step 1 and define a
morphism ? : v®(AW, d) —> (Q®N, d) of [AW, d)-modules by setting ?(?®?) =
(-1)***? .ZQm We shall show that ?? = id.
Since ? and ? are AW-linear it is enough to show that ?\(? ®\) = ? ® 1. But
?\(? ®\) — tv®l, some t G 3c, for reasons of degree. Moreover ?\(? ® 1) = ?zQ
differs from azc by a coboundary in Av <g> AW, because ? ~ ? (Step 3). Since
? restricts to 7 in Q (Step 2) and since H(-j)lq = [v ® 1] (Step 1) it follows
that t[v ® 1] = [v ® 1] in H(Av ® AW, d). Since (?? ® AW,d) is a minimal
Sullivan algebra ? ® 1 cannot be a coboundary; i.e. [v ® 1] ? 0 and t = 1. Thus
?\(? ®\)-?®\ and ?? = id.
Finally, recall the (Av ® AW, (i)-quasi-isomorphism
? : (Q, d) ~ ? ® (AW/A>mW, S)
defined at the start of the proof of the proposition. It extends to the quasi-
isomorphism
? ® id : (Q ® N, d) —> ? ® (AW/A>mW, d) ® (N, 0)
since ?(vQ) = ? · ?(?)) = 0. Moreover we can make ? ® (AW/A>mW, d) ® (N, 0)
into a (AW, d)-module , and ? into a morphism of (AW, d)-modules, by setting
? -(? ® ? ® an) = (-l)dee* X;(-l)p ( P + n ) (<???) ? ® an+p .
P=o \ ? /
Thus altogether we have the diagram of morphisms of (AW, d)-modules
? ® (AW, d) (Q ® N, d) ^ ^ ? ® (AW, d)
~ ?.®id
v ® (AW/A>mW, d) ® (N, 0) ,
with ?? = id.
Since Av ® AW is a minimal Sullivan algebra, imdCl® A^2W + v® A+W.
Thus ? preserves A+W. It follows that with respect to the module action just
defined,
W · (v ® AW/A>mW ® N) C ? ® A+W/A>mW ® ? .
430 31 The homotopy Lie algebra and the holonomy representation
Hence A>mW · (v ® AW/A>mW ® N) = 0. Now the Corollary to Hess' theo-
rem 29.9 asserts that cat(ATV, d) <m. D
proof of Theorem 31.11: Recall that V is equipped with a basis (vt). Di-
viding by the elements v\,... , u;_i gives a quotient Sullivan algebra of the form
(AVi ® AW,Di) in which Vi,Vi+i,... project to a basis of Vj·. Moreover the
Mapping theorem 29.5 asserts that
cat(AV, ® AW, D{) > cat(AFi+1 ® AW, Di+i) > > cat(AW, d) .
Thus Theorem 31.11 follows from the assertion:
If Xi G Leven and if adxj is locally nilpotent and ?(?{) is locally conilpotent,
then
cat(AVj ® AW, Di) > cat(AVi+i ® AW, Di+1) + 1 .
To verify this, simplify notation by writing Vi — v, Vi+1 = U, #* = ?? and X{ =
x. Denote D{ by D and Di+l by ~D. Thus the Sullivan algebra (AVi ® AW, D{)
has the form (Av ® AU ® AW, D). The homotopy Lie algebra of (?? ® ??7, D) is
the subspace I C L spanned by the Xj, j > i. In particular adx : / —> I is the
restriction of ad X{ : L —> L and hence is locally nilpotent.
Since ? has even degree, ? has odd degree. Thus the differential in ?? ® ??7 ®
AH'* is gi\7en by Dv = 0 and
D(\ ® ?) = 1 ® ?)? + ? ® ?? , ? G AU ® Aiy ,
where O is a derivation in (??/???7, D). Lemma 31.9 asserts that ?(?) is locally
conilpotent, and thus Proposition 31.13 applies to give cal(Av ® AU® AW, D) >
cai(AU®AW,~D) + l. D
(e) Examples.
Example 1 The fibration ? : S4m+3 —l· MPm.
The unit sphere S3 of the quaternions El acts freely by right multiplication
on the unit sphere S4m+3 of Hm+1 (= M4m+4). This is the action of a classical
principal S3 bundle (§2(a)) p : S4m+3 —> MPm, with base space the quaternionic
projective m-space.
The long exact rational homotopy sequence for this fibration reduces to
ir4rn+3(S4m+3)®Q^K4m+3(MPm)®® and ?4 (MPm)®Q A n3(S3)®Q .
Thus dim ?, (MPm) ® Q < oo and dimwodd (HPm) ® <Q> = 1. Moreo\'er, the
holonomy representation is automatically locally nilpotent (but not trh'ial) since
the fibre has finite rational homology.
Thus all the hypotheses but one of Theorem 31.10 are satisfied: <9, ® Q ? 0.
Since cat0 S4m+3 < cat0 S3 + dim ???? (MPm ) ® Q the conclusion of the theorem
fails too, which shows that the hypothesis ?, ®Q = 0 is necessary. (Note, howe\rer
that it intervenes only at the end, in Step 4, of the proof of Proposition 31.13).
D
Rational LS-Category 431
Example 2 The fibration associated with S3 V S3 —> S3.
Convert projection from S3 V S3 to the first factor to a fibration ? : X —> S3
with X ~ S3\JS3-- Since ? is not a rational homolog}' equh'alence the fibre, F, has
non-tri vial rational homology and so cato F >1 = cat0 „Y, and the conclusion of
Theorem 31.10 fails.
Here all the hypotheses hold, however, except for the hypothesis that the
holonomy representation is locally nilpotent. This must therefore fail, and the
example shows it is a necessary hypothesis. D
Example 3 Derivations in commutative cochain algebras.
Suppose ? is a derivation of even (negative) degree in a commutative cochain
algebra (A, dA) such that A0 = h and Hl{A) — 0. Then we may construct
the commutative cochain algebra (Av <g> A, d), with degu + deg# = 1 by setting
dv = 0 and da — dAa + ? <g> ??, a G A.
Now let (AW, d) be a minimal model of (A, d). Then (Av®A, d) has a Sullh'an
model of the form (Av ® AW, d) with ?? = 1 <g> ?? + ? <g> ?'?. If (?? <g> AW, d) is
not a minimal Sullivan algebra then ?' restricts to a non-zero Gottlieb element
jydegt;-i __j. A and Proposition 29.8(_ii) asserts that cat(AW,d) = oo. On the
other hand, the isomorphism H(AW,d) = H(A,dA) identifies ?(?') with ?(?).
Thus if ?) Im H(9)k — 0 we can apply Proposition 31.13 to conclude that
k
f < catiAu <g> AW, d) — 1 , if (?? ® AM7, d) is minimal.
cat(AW, d) is <
I oo , otherwise.
An interesting special case of the above is an arbitrary Jfc[0]-module M con-
centrated in degrees > 2, from which we construct (A, cU) by setting ^1 = k®M,
dA = 0 and M ¦ M = 0. In this case if ? is locally conilpotent then
caA(Av®AW,d) = 2 .
In fact since (AW,d) ~ (A,0) and niL4 = 1 it follows that cat(AW,d) = 1
(Proposition 29.3) and hence cat(Au ® AW, d) > 2. On the other hand, (Av <g>
AW, d) -=> (Av <g> A, d) and nil (Au ®A) = 2, so cat(Au ® AH7, d) < 2. D
Example 4 ? fibration X —> CPm ????? ^ere 53 and cat0 X = 2.
Let S1 act on 52m+1 by complex multiplication: S1 is the unit circle of C and
52m+1 is the unit sphere in <Cm+1. This is the action of a principal S^bundle,
52m+1 —> €Pm (§2(d)). For m = 1 we have the action of S1 on S3 and the
associated bundle (§2(e))
p:X = S3 xSi 52m+1 —> CPm
is a Serre fibration with fibre S2.
Since ?, (CPm)®Q is concentrated in degrees 2 and 2m+1 it follows for degree
reasons that the rational connecting homomorphism is zero. Since the fibre has
432 31 The homotopy Lie algebra and the holonomy representation
finite dimensional homology the holonomy representation is by locally nilpotent
transformations. Thus Theorem 31.10 applies and gives cato X > cat0 53 + l = 2.
But \ve may also regard X as the total space of a Serre fibration X —> S2
with fibre 52m+1, so that cat0Ar < 2 (Proposition 30.7). Altogether we have
cato X = 2. ¦ D
(f) Iterated Lie brackets.
Let Lx be the homotopy Lie algebra of a simply connected topological space
X with rational homology of finite type. Apply Theorem 31.10 to the fibration
? -?4 ? to obtain
Theorem 31.16 If cato X = m there are at most m linearly independent ele-
ments Xi € (Lx)even such that adxi is locally nilpotent.
In particular if cato X is finite then for each non-zero ? € (Lx)even of suf-
ficiently large degree there is some y € Lx such that the iterated Lie brackets
[x, [x[x,..., [x,y] ¦¦¦]]] are all non-zero.
Similarly, if L is the homotopy Lie algebra of a minimal Sullivan algebra (AV, d)
with V = V-2 a graded vector space of finite type, then Theorem 31.11 applied
to the relathre Sullivan algebra (AV, d) -^-> (AV, d) ghres
Theorem 31.17 i/cat(AV, d) = m there are at most m linearly independent
elements x^ € Leven such that ad X{ is locally nilpotent.
Example 1 A space with e0X = 2 and cato X = oo.
In Example 6, §12(d), we constructed a minimal Sullivan model (AV,d) such
that d : V —> A3V and every cocycle in A-3V is a coboundary. Moreover
V = V-2 and has finite type and it is immediate from the construction that we
may choose Vodd to be infinite dimensional.
Because every cocycle in A-3V is a coboundary, e(AV, d) < 2. Because there
are no coboundaries in A2V, e(AV,d) = 2. Because d: AkV —> Afc+3V the
Milnor-Moore spectral sequence collapses at the ^-term. On the other hand,
the homotopy Lie algebra is abelian, because the quadratic part of d is zero.
Thus Theorem 31.18 shows that cat(AV,d) = oo.
In particular, if X is the geometric realization of (AV,d) (§17) then
cat0 X = cat (Ay, d) = oo and e0X = e(AV, d) = 2 . D
Finally recall that the construction of (AV,d) begins \vith a graded subspace
? C V such that d{Z) = 0, and that V = ? ® W with d injective in W.
Let / be the ideal A-2V © W, and let (A,d) be the sub cochain algebra given
by A = ??2*?. The inclusion (A,d) —> (? ? lk,d) is a quasi-isomorphism.
k
Moreover, dividing by (A+J and by a complement of kerd in A2V defines a
quasi-isomorphism (A, d) -^ ? (A) and shows H+(A) · H+(A) = 0.
Rational LS-Gategory 433
The surjection (AV, d) —> (AV/I,d) is a commutative representative for a
continuous map q : X —> \J Sna, where Sna corresponds to a basis element of
a
? with degree na. Moreover (/ 0 it) is a commutative model for the cofibre of
q as follows from a simple calculation using the Remark after Proposition 13.6.
In view of the observations above / ? it is the commutative model of a wedge of
spheres (Example of §12(a)) and so we have constructed a cofibration
Exercises
1. Let F -> X -> S2n+1 be a fibration. Suppose that F has the homotopy
type of a simply connected CW complex of finite type and that Ht(QS2n+1)
acts trivially on H*(F). Show that the induced map Ht(F;Q) -> H*(X;Q) is
injective.
2. Let / : X = (S3 x S3) V (S5 x S5) -> S3 be the continuous map whose
restriction to S3 x S3 is projection onto the first factor, and that restricts to
the trivial map on S5 x S5. Denote by F the homotopy fibre of /. Prove that
catX = cato-F = 2. Prove that H*(F;Z) is not a free ii,(fiS3)-module, nor a
locally nilpotent one.
3. Let ? : X -> 52n+1 be a fibration with simply connected fibre. If n2n+i(S2n+1)
acts locally nilpotently on H*(F;k) and if H*(X;k) is finite dimensional, prove
that H*{F;k) is finite dimensional.
4. We consider the rational fibration V™=1Sq -> A" -> 5??+1 whose commutative
model is given by (?? <g> (?,??,... ,an),d) with at-ay = 0 and d(di) = ???-?-
Prove that: adx.(az·) = ??·+? ,... ,adx(an) = 0. Using Jessup's result, prove that
cat0A/' = 2.
5. Prove that the fibration F -? ? -i S2n+1 , ? > 1, admits a Lie model that is
a short exact sequence of differential graded Lie algebras
0 -> L(r(x) <8> MO -»· L(Qa; © W) -»· L(x) -»· 0.
Interpret the holonomy operation as multiplication by x.
6. Denote by ?? the subspace of Lx generated by the Engels elements of
7?.? ® Q. Prove that ^(X) C ?*·. Construct an example with GQ(X) ?
Ex ? Lx.
7. Let F 4 ? -> ? be a fibration with dim ?»(?) (g) Q < oo and ker^»(j) <8>
Qjcvcn _ q Suppose that H,((W;Q acts locally nilpotently in H,(F;Q), and
prove that
Part VI
The Rational Dichotomy:
Elliptic and Hyperbolic Spaces
and
Other Applications
32 Elliptic spaces
In this section the ground ring is an arbitrary field k of characteristic zero.
A simply connected topological space X is called rationally elliptic if it satisfies
the two conditions:
dim#„(X;Q) < oo and dimir*{X) ® Q < oo .
By analogy a minimal Sullwan algebra (AV,d) is elliptic if both H(AV, d) and
V are finite dimensional. If (AV, d) is a minimal Sullivan model for a simply
connected space X then
H*(AV,d) = H*(X;k) and V = Romz(n,(X),k)
(Theorem 10.1 and Theorem 15.11). Thus X is rationally elliptic if and only if
its minimal Sullivan models are elliptic.
Rationally elliptic spaces occur naturally; for instance the classical homoge-
neous spaces G/K of differential geometry are rationally elliptic. Howe\'er, the
'generic' space with finite homology is not rationally elliptic; for example, in this
section we shall see that the homotopy groups of rationally elliptic spaces satisfy
very stringent restrictions.
Indeed, suppose X is rationally elliptic and let ?? be the maximum degree
such that Hnx (X;Q) ? 0. In Theorem 32.6 we shall show that if (xt) is a basis
of 7TOdd(-20 ® Q and if (yj) is a basis for 7reven(J\f) <g> Q then
• ? degXi < 2?? — 1 and ?deg?/7· < ??,
so that, in particular,
• tti(X) ® Q = 0 , i > 2nx and dim^(A') ® Q < nx.
We also obtain a formula for ??:
Vi - 1)·
i j
Finally, in Proposition 32.10 we shall show that
• dim^dd(X)®Q-dim^ven(X)®Q> 0 and J2(-iy dimH^X;^) > 0,
i
with equality holding on the left if and only if it fails on the right.
These various inequalities have interesting geometric properties. For example
• If X is rationally elliptic then
dim TToddpf) ® Q < cat0 X ,
and (Allday-Halperin [3])
The Rational Dichotomy 435
• // an r-torus acts smoothly and freely on a rationally elliptic closed mani-
fold X then
r < dim 7rodd(A') ® Q - dim 7reven(A') <g> Q .
In particular, if X — G IK with G and ? compact simply connected Lie
groups then
r < rank G — rank if .
Most of these results are obtained by reduction to the case of pure Sullivan
algebras (AV, d); these are the finitely generated Sullh'an algebras in which d =
0 in Veven and d : Vodd —> AVeven. Cochain algebras of this special form
were introduced by H. Cartan in [33] for the computation of the cohomology of
homogeneous spaces; in retrospect these can be seen to be the first examples of
Sullivan algebras. The properties of pure Sullivan algebras as established in (a)
and (d) below are largely due to Koszul [102], and indeed they are often referred
to as Koszul complexes.
This section is organized as follows:
(a) Pure Sullivan algebras.
(b) Characterizations of elliptic Sullh'an algebras.
(c) Exponents and formal dimension.
(d) Euler-Poincare characteristic.
(e) Rationally elliptic topological spaces.
(f ) Decomposability of the loop spaces of rationally elliptic topological spaces.
(a) Pure Sullivan algebras.
Given a Sullivan algebra (AV,d), write Veven = Q and Vodd = P, so that
Ay = AQ <g> AP. Recall that (AV, d) is called pure if V is finite dimensional and
if d = 0 in Q and d(P) C AQ. If (AV, d) is a pure Sullivan algebra, then d is
homogeneous of degree — 1 with respect to wordlength in P.
0 <— AQ <— \Q ® ? < 4— AQ ® i\kP < .
Thus if Hk(AV,d) is the subspace representable by cocyles in AQ®A*P we have
H(AV,d) = ® Hk(AV,d)i this will be called the lower grading of H(AV,d).
k
Note that d(AQ ® P) — AQ - d(P) is the ideal in the polynomial algebra AQ
generated by d(P). Thus
H0(AV,d) = AQ/AQ-d(P) .
Proposition 32.1 If (AV, d) is apure Sullivan algebra then H(AV,d) is finite
dimensional if and only if Ho(AV; d) is finite
436 32 Elliptic spaces
proof: Since AV is a finitely generated module over the (noetherian) polyno-
mial algebra AQ any submodule is also finitely generated. Since d{AQ) — 0,
kerd is a AQ-submodule of AV; hence it is finitely generated. Thus H(AV, d) is
finitely generated as a module over the subalgebra H0(AV,d). The Proposition
follows. D
Consider again a pure Sullivan algebra (AV, d) as above and suppose 2??,...,
2aq are the degrees of a basis (yj) of Q and 2bx - 1,..., 2bp - 1 are the degrees
of a basis (xi) of P. (Thus dim Q = q and dim ? = p).
Proposition 32.2 Suppose H(AV,d) is finite dimensional and let r and ? be
the maximum integers such that Hn(AV,d) ? 0 and HT(AV,d) ? 0. Then
(i) Hn(AV,d) is a one-dimensional subspace of Hr(AV,d).
(ii) r = dim ? — dim Q; in particular, dim ? > dim Q and equality holds if and
only if H(AV,d) = H0(AV,d).
(in) ? = ?B^-1)-
i i
proof: Define a graded \'ector space Q by Q = Ql+1 and denote the cor-
respondence between elements by y «-» y. Extend (AV, d) to a pure Sullivan
algebra (AQ ® A(P <g> Q), d) by setting dy = y, y G Q. This can be written as
(AQ <g> AQ <g> ??, d) and (AQ <g> AQ, d) is contractible (§12(b)). Thus division by
Q and Q defines a quasi-isomorphism (AQ ® AQ <g> AP,d) ^> (??, 0), whence
#sm (AQ «8» ?? «8» AQ, d) = (AsP)m .
Let k be the largest integer such that Hr(AV,d) ? 0. It is easy to construct
an ideal / C AQ <g> ?? such that: / is preserved by d, H (I, d) = 0, / = ? / ?
s
(AQ <g> ?5?) and / D (AQ <g> A5P)^ if s > r or if s = r and I > k. Let (A, d) be
the quotient cochain algebra (AQ <g> AP/I.d) and note that A = ®AS, where
5
As is the image of AQ <g> A5P, and that A+ -A* C A? ¦ A* + A>r = 0. By
Lemma 14.2 the quasi-isomorphism ? : (AQ ® AP,d) —> (A,d) extends to a
quasi-isomorphism
(? ® id) : (AQ ® ?? ® AQ,d) ^> (A ® AQ,d) ,
where dy = ?dy. Thus if iiis(^4 ® AQ) is the space represented by cocycles in
? Ai ® AJ'Q, we have
fism(^ ® AQ) ^ H™(AQ ® ?? ® AQ) = (AsP)m .
Now let ? G if be a cocycle representing a non-zero cohomology class. Since
A+ ¦ A*t = 0, and since dy~i G A+, a®y~iA---Ayq is a cocycle in ? <g> AQ. It
The Rational Dichotomy 437
cannot be a coboundary, since (A®AQ)r+q+1 = 0. Thus it represents a non-zero
cohomology class of lower degree r + q, which is obviously the maximum lower
degree possible. Since dim ? = ? this gives an inclusion
H${A) ® ft ? · · · ? yq C Hr+q(A ® AQ) ? APP .
In particular r + q = ? and r = p- q = dim ? — dim Q. Since dim APP = 1 we
have dim H^(A) — 1 and, if x\,..., xp is a basis of P,
fc =
Finally, let ? be the largest degree such that HN(A ® AQ) ? 0 and let
J C AQ ® ?? be an ideal of the form (AQ ® AP)>n © Jn chosen so that
H(J,d) = 0. Set ? = AQ ® AP/J. Then the identical argument gives
/i ? · · · ? yq C ^^E ® AQ) S APP .
This shows that ? — k, which completes the proof. ?
Finally, let Q be a finite dimensional graded vector space concentrated in
even degrees. A regular sequence in the polynomial algebra AQ is a sequence
of elements «i,...,uTO in A+Q such that u\ is not a zero divisor in AQ and
(for i > 2) the image of Ui is not a zero divisor in the quotient graded algebra
On the other hand any sequence ulr..,um of elements in A+Q determines
the pure Sullivan algebra (AQ ® ?(??,... ,xm), d) defined by dxi = Ui and we
have
Proposition 32.3 The sequence U\,... ,um is regular if and only if
H+(AQ®A(xu...,xm),d) = 0.
proof: We show first that if H+(AQ<8>A(xi,... ,xm-i)) = 0 and if ui,..., um_i
is a regular sequence then H+(AQ<8>A(xi,.. ¦ ,xm),d) = 0 if and only if ui,... ,um
is a regular sequence. Denote by / the ideal in AQ generated by «i,..., um-i·
Under the hypotheses above a quasi-isomorphism ? : (AQ ® A(a^i,... ,a;m_i), d)
—> AQ/I is defined by: ??? — 0 and, for a G AQ, ?? is the image of ? in AQ/1.
Then (Lemma 14.2) ?®??: (AQ <8> ?(??,... ,xm),d) -=> (AQ/I <g> A:rm, d) is a
quasi-isomorphism, where dxm = ??-m-
Let ? C AQ/I be the subspace of elements u such that u · ipum = 0. Then
H(AQ/I <g> Axm,d) = AQ/(u\....,um) © (? ® xm). Since the isomorphism
?(?®??) preserves lower degrees (clearly) we have Ar>2(AQ®A(a;i,..., xm), d) =
0 and Hi(AQ® A(x\,... ,xm)) — K®xm- Since ? = 0 if and only if ui,... ,um
is a regular sequence, this is equivalent to H+ (AQ ® A(^!,... ,xm)) = 0.
Finally, if m, ¦ ¦ ¦ ,um is a regular sequence so is «i,... ,um_i and so by in-
duction H+(AQ® A{xi,...,xm-\)) = 0. Thus H+(AQ ® A(xu ... ,xm)) = 0.
438 32 Elliptic spaces
Conversely, suppose H+(AQ ® A(:ri,.. .,xm)) = 0. For any k, let ? G AQ <g>
Ak(xi,. .. ,xm-i) be a cocycle representing a non-zero class of least degree in
Hk(AQ ® A(:ri,... , ?m_i)). A simple calculation shows ? is not a coboundary
in AQ® A(xi,...,xm)· Thus k = 0 and H+(AQ <g> ?^?,... ,xm_i)) = 0. By
induction, ui,... ,um-i is a regular sequence and so by the argument above, so
is«i,...,um. D
(b) Characterization of elliptic Sullivan algebras.
Suppose (AV, d) is a Sullivan algebra in which V is finite dimensional. Write
yodd = ? and yeven _ q^ so that AV = AQ <g> AP is the tensor product of
the polynomial algebra, AQ and the exterior algebra, AP. Then bigrade AV
by setting (AQ ® AkP)n = (AV)k+n>~k and filter AV by setting F"(AV) =
(AV)^P'*.
Since the differential increases degree by one and decreases the wordlength in
? by at most one it preserves the filtration. Thus (AV, d) is a filtered cochain
algebra, with which there is then a naturally associated spectral sequence of
cochain algebras, called the odd spectral sequence for the Sullivan algebra.
It is immediate from the definition that the first term of the odd spectral
sequence has the form
where da is characterized by:
dff(Q) =0 ,?? :P —>AQ and d - d„ : ? —> AQ ® A+P .
Thus (AV, da) is a pure Sullivan algebra and (in the notation of §32(a))
E™ = Hp_+qq{AV,da) .
We call (AV, da) the pure Sullivan algebra associated with (AV, d).
Note also that Fp((AV)n) = 0, ? > n + dimP. Thus the odd spectral sequence
is convergent to H(AV, d).
Proposition 32.4 Let (AV, d) be a minimal Sullivan algebra in which V is
finite dimensional and V = V-2. Then the following conditions are equivalent:
(i) aixnH{AV,d„) < oo.
(ii) dimH(AV,d) < oo.
(Hi) cat(AV,d) < oo.
proof: Since the odd spectral sequence converges from H(AV, da) to #(AV, d)
the implication (i) =?· (ii) is immediate, while (ii) =$» (iii) follows from Corol-
lary 1 to Proposition 29.3. To prove (iii) =? (i) let ??,.. .,xn be a basis of V
such that degzx < deg^2 < ··· and dxi G A(x\,...,Xi-{). We show first by
induction on i that if dega^^ is even then for some JVj, xi ' = da^i.
The Rational Dichotomy 439
For this, divide by the ideal /* generated by x\,... ,Xi-\ to obtain a quotient
Sullivan algebra (AV,d). Then Xi,...,xn project to a basis (xj) of V, and
dxi = 0. On the other hand, by the Mapping theorem 29.5 (AV, d) has finite
category. Thus there is an element ? G A(xi,... ,xn) such that d? = xf (some
N), where ? is the image of ? in AV. Since deg ? is odd we have ? = ?? + ?3+· · ·
with ?^· ? AjVodd ® AVeven. It follows that (djj, = ??, which implies that
Define elements yj G V by setting yj = Xj if dega^j is even and yj = 0 if
degxj is odd. Since ?? G Vodd ® AVeven it follows that ???? - ?? =
j G AVeven. The induction hypothesis gives yj > = da^j, j < i. Since
daClj = 0 follows that some power of Xi is a d^-coboundary. This closes the
induction.
As in §32(a) put Q = Veven and ? = Vodd. We have just shown that for
a basis yj of Q some power y · J is a da-coboundary. It follows that Hq(AQ ®
AP,dc) = AQ/AQ -da(P) is finite dimensional. Hence H(AQ <g> AP,dc) is also
finite dimensional (Proposition 32.1). D
Example 1 ?(?2,^3,U3,64,^5,^7; da = dx = 0, du = a2, db = ax, dv =
ab — ux, dw = b2 — vx).
Here subscripts denote degrees. The differential da is given by daa = dab =
dax = 0, dau = a2, dav — ab, daw = b2. Thus in H(AV,da) we have [a]2 =
[b]2 - 0 and so (AV, d) is elliptic. D
Example 2 Adding variables of high degree.
Suppose (AV", d) is a minimal Sullivan algebra in which V — V-2 and V is finite
dimensional. It is an interesting question of Anick whether, given an arbitrary
positive integer n, there is an elliptic Sullivan algebra of the form (AV" ® AW, d)
with W concentrated in degrees > n.
For example, consider the Sullivan algebra (subscripts denote degrees)
?(?2, b2, X3, y3,z3, C4; da = db = 0, dx — a2, dy = ab, dz = b2, de = ya — xb) .
It is not elliptic because dac = 0 and no power of c is a d<r-coboundary. On the
other hand, if we add odd degree variables u, ? and w with
du - cna - ncn~1xy, dv = cn+1b - (n + \)uyb - (n + \)cnxz + (n+ \)uaz
and dw = c2n+2 - 2(n + l)cn+1uy - 2(n + \)vua + 2(n + l)vckx
then we obtain an elliptic Sullivan algebra, since daw = c2n+2. ?
Again let (AV, d) be a minimal Sullivan algebra and denote by k[z] the poly-
nomial algebra in a single variable, 2, of degree 2. (Note Jk[z] = Az, but we wish
to emphasize that this is a polynomial algebra.)
Proposition 32.5 Suppose V is finite dimensional and V = V-2, and suppose
Jk is algebraically closed. Then (AV, d) is elliptic if and only if every morphism
? : (AV, d) —> (J;[z],0) is trivial.
440 32 Elliptic spaces
proof: Suppose (AV, d) is elliptic and let x\,..., xn be a basis of V such that
dxi G ?(?!,... ,?;_i). Given </? : (AV, d) —» (jfo[z], 0), suppose by induction that
??? = 0, j < i. Then ? factors to give a morphism ? : (A(x{,... , ?n),d) —>
(]k[z],0) from the quotient Sullivan model. If degzj is odd then ??? = 0. If
deg ?* is even then, since this quotient model has finite category (Theorem 29.5),
xf = ??, some JV. Hence (???)? = ?(?^) = ??? = 0. It follows that ??? = 0.
Thus ? is trh-ial.
Conversely, suppose (AV, d) is not elliptic. Then by Proposition 32.4, H{AV, dc)
is infinite dimensional, and hence so is H0(AV,da), by Proposition 32.1. This
algebra has the form A(j/i,... ,yq)/I where / is generated by polynomials /,·,
1 < i < p. Since it is infinite dimensional there is some yj such that y*j $ I for
all A;.
Extend the polynomial algebra A(y1;... ,yg) to a polynomial algebra
AB/1, ¦ · ·, Vq, u) with deg u = - deg yj. Then the ideal generated by A,..., /p
and by pjU — 1 is properly contained in A(y\,... ,yq,u). Thus the Hilbert
Nullstellensatz asserts the existence of scalars h,... ,tq, t G Jk such that each
fi(h, ¦ ¦ ¦ ,tq) = 0 and such that tjt = 1. In particular, tj ? 0, and a non-
trivial morphism ? : A(yi,... ,yq)/I —> h[z\ is given by yz- 1—> t{zai, where
degyi = 2at.
Finally, let J C AV be the ideal generated by Fodd and by Imd. Then
AV/J = AVeven/AVeven-da(Vodd) = A(yu...,yg)/I, and a non-trivial mor-
phism (AV,d) —> fo[z] is obtained by composing (AV,d) —> (AV/J,0) A k[z\.
D
Example 3 Algebraic closure of k is necessary in Proposition 32.3.
Indeed if Jk = Q the Sullivan algebra A(a2.&2)?3;cte = a2 + b2) admits no
non-trivial morphism to Q[z], since we would have a \—> az, b <—>¦ ?? with
?, ? G Q satisfying a2 + ?2 = 0. D
Example 4 (Lechuga and Murillo,[106]) ?-colourable graphs.
To each finite graph with vertices vj and edges e% and for each integer ? > 2
associate a pure Sullivan algebra (AQ <g> ??, d) as follows: Q is concentrated in
degree 2 and has a basis yj corresponding to the vertices Vj\ ? is concentrated
in degree 2n — 3 and has a basis Xi corresponding to the edges e*; finally dxi =
^Ty^y"-, where yk and y^ correspond to the endpoints of e*.
The graph is ?-colourable if each vertex can be assigned one of ? distinct
colours so that vertices connected by an edge have different colours and, as
slKnvn in [121]:
A finite connected graph is n-colourable 4=^ dim H(AQ ® ??, d) = 00.
Indeed we lose no generality in assuming k is algebraically closed. If the graph
is n-colourable identify the colours with the distinct nth roots of unity wa and
note by wQ(j) the colour of the vertex Vj. If wa and W? are distinct nth roots of
unity then ^,w^w^~s = w^ — W?/wa — W? = 0, and so a non-trivial morphism
(AQ ® ??, d) —> k[z] is given by yj 1—> vja(j)z and Xi 1—> 0.
The Rational Dichotomy 441
Conversely, given a non-trivial morphism ? : (AQ ® ??, d) —> ]k[z] note that
if yic and ye correspond to the vertices of ei then 0 = ip{dxi){ipyk — (pye) =
s&yk - vw) = (</>?/fc)n - {???)?· Since the graph is connected
5
there is a single scalar ? such that (y>yj)n = Xzn, and since some c>yj ? ?, ? ? 0.
Choose an nth root of ?, ?, and define wa(j) by ipyj = wa^\z. This n-colours
the graph. D
(c) Exponents and formal dimension.
Fix an elliptic Sullivan algebra (?V, d) and bases y\,..., yq of Veven and
??,. ..,???? Vodd. The sequences ??,..., aq and bi,...bp defined by deg yj = 2aj
and degZj = 26Z- — 1 are independent (up to permutation) of the choice of basis.
Definition The integers ??,..., ?, and b\,..., bp are respectively the even and
the odd exponents of (AV, d).
The formal dimension of (AV, d) is the largest integer ? (or oo) such that
??(??,?)?0.
In this topic we prove
Theorem 32.6 (Friedlander-Halperin [61] Suppose (AV, d) is an elliptic Sulli-
van algebra with formal dimension ? and even and odd exponents ??,..., aq and
bi,... ,bp. Then
? g
g
(ii) 2_\2aj < n-
?
(iii) y^Bbi - 1) <2n- 1.
(iv) dimyeven < dimV01111 < cat(Ay,d).
Corollary 1 // (AV, d) is an elliptic Sullivan algebra of formal dimension ?
then V is concentrated in degrees < 2n — 1, and at most one basis element ofV
can have degree (necessarily odd) > n.
Corollary 2 If (AV, d) is an elliptic Sullivan algebra of formal dimension ?
then dim y < ?.
proof: We have ? = ? Bbt - 1) - ? BaJ - 1) > ? B&t - 1) + Q > ? + V,
i=l j=l i=l
where ? = dim Vodd and q = dim Veven. ?
442 32 Elliptic spaces
The proof of Theorem 32.6 depends on a reduction to the pure case (Lemma
32.7) and a characterization of exponents (Proposition 32.9).
Consider then an elliptic Sullivan algebra (AV, d). By Proposition 32.4 the
associated pure Sullivan algebra (AV, ??) also has finite dimensional cohomology.
Lemma 32.7 (AV, da) and (AV, d) have the same formal dimension.
proof: We argue by induction on dimV. Write (AV, d) as a relative Sullivan
algebra (Av ® AW, d) in which V = hv ? W and ? is an element in V of minimal
degree. The Mapping theorem 29.5 asserts that the quotient Sullivan algebra
(AW,d) has finite category; hence it too is elliptic (Proposition 32.4).
Next observe that if m is the formal dimension of (AW, d) and ? is the formal
dimension of (AV, d) then
{m + deg ? if deg ? is odd
, , C2·8)
m — deg ? + 1 if deg ? is even.
Indeed if deg ? is odd (and therefore > 3) this follows from the long exact coho-
mology sequence associated with
0 —> ? <8> (AW, d) —» (Av <8> AW, d) —» (AW, d) —» 0 .
If deg ? is even extend (AV, d) to (AV <8> Av,d) by setting dv = v, and use the
long exact cohomology sequence associated with
0 —» (AV, d) —» (AV <g> ??, d) —» (AV, d) <g> ? —> 0
to conclude that formal dimension (AV®Av,d) = n+degu = n+degf —1. Then
write (AV®Av,d) = (Av<S>Av<S>AW, d) and observe that because H(Av<S)Av,d) =
Jk the surjection (AV <8> ??, d) —> (AW, d) is a quasi-isomorphism.
This proves C2.8). The same argument applies to the relative Sullivan algebra
(Av <8> AW, da), and here the quotient Sullivan algebra (AW, da) is just the pure
Sullivan algebra associated with (AW,d). By induction (AW,d) and (AW, dc)
have the same formal dimension. Thus formula C2.8) for (AV, d) and for (AV, da)
shows these have the same formal dimension too. ?
Our next step is to derive the fundamental property enjoyed by the exponents
of an elliptic Sullivan algebra.
Proposition 32.9 (Priedlander-Halperin [61]) Suppose a\,..., aq and b\,..., bp
are the even and odd exponents of an elliptic Sullivan algebra (AV, d). Then for
any integer s A < s < q) and any subsequence a^,...,ajs of even exponents,
there are at least s odd exponents bi that can be written in the form
The Rational Dichotomy 443
where the k\ are non negative integers (depending on bi) and Y^k\ > 2.
?
proof: Since the associated pure Sullivan algebra is also elliptic (Proposi-
tion 32.4) we lose no generality in assuming that (AV, d) is itself pure. Re-index
the even exponents so that the subsequence in question is ??,..., as. Let (yy)
and (??) be bases of Veven and Vodd chosen so degyy = 2ay and degXi = 2bi — 1.
Divide by the yy, j > s, to obtain a pure elliptic Sullivan algebra of the form
(A(yi,... ,ys) ® A{xu ... ,xp),d).
Next, renumber the basis xt so that dxt ^ 0, 1 < i < r and dxi = 0, i > r.
Then our pure Sullivan algebra has the form (A(yi,... , ys) <g> A(a;1,... ,xr),d) <g>
{h(xr+i,... ,Xp), 0) and so (A(yi,... , ys) <g> A(a;i,... ,xr),d) is elliptic. By Propo-
sition 32.2 (ii), r>s.
On the other hand, for i < r, dxi is a non-zero polynomial in the yy with no
linear term, and so it contains a term of the form yf' · · ·y*5 with Y^k\ > 2.
f \
Thus 2bi = degXi + 1 = ? kx degyA = 2
?=? \?=?
Remark In [61] it is shown via a difficult argument from algebraic geometry
that any pair of sequences ax,..., a, and b\,...,bp satisfying the conclusion of
Proposition 32.9 are the even and odd exponents of an elliptic Sullivan algebra.
Thus this condition precisely characterizes the possible degrees of a basis for V
in an elliptic Sullivan algebra (Ay, d). D
proof of Theorem 32.6: In view of Lemma 32.7 we may suppose (AV, d) is
pure. Thus (i) is just Proposition 32.2 (iii). Now order the exponents so that
ci > a2 > ¦ ¦ ¦ > aq and b\ > b% > ¦ ¦ ¦ > bq. At least s of the bi are non-
trivial non-negative integral combinations of a\,...,as (Proposition 32.9). In
particular, at least s of the bi satisfy bi > 2as. This implies that bs > 2as. Thus
by(i)
Similarly,
? =
?
and so 2n - 1 > ? {2bi - 1).
i=l
This proves (i)-(iii). Proposition 32.2 (ii) asserts that dim Veven <
which is the first inequality of (iv). Finally, the homotopy Lie algebra L of
(Al7, d) is finite dimensional, since sL is dual to V. Thus adz is locally nilpotent
for all ? ? L and so Theorem 31.17 implies that cat(AV, d) > dimLeven (=
dimVodd). D
444 32 Elliptic spaces
(d) Euler-Poincare characteristic.
Recall (§3(e)) that the Euler-Poincare characteristic xm of a finite dimensional
graded vector space is defined by ?? = ?(-1)' dim Mi = dim Aieven-dim Modd.
If M is equipped with a differential, d, then xm = XH(M,d), as follows from
elementary linear algebra.
The purpose of this topic is to prove
Proposition 32.10 Let ? be the Euler-Poincare characteristic of the cohomol-
ogy of an elliptic Sullivan algebra (AV,d). Then
? > 0 and dim Fodd - dim V'even > 0 .
Moreover, the following conditions are equivalent:
?) X > 0-
(ii) H(AV,d) is concentrated in even degrees.
(Hi) H(AV, d) is the quotient A(y\,..., yq)/(u\ , - -., uq) of a polynomial algebra
in variables (yj) of even degree by an ideal generated by a regular sequence
(iv) (AV,d) is isomorphic to a pure Sullivan algebra (AQ <25 AP,d) in which
Q z= Qeven, ? z= podd and d maps a basis of ? to a regular sequence in
AQ.
(v) dim y°dd - dim Veven = 0.
The proof of the Proposition relies in part on another invariant of pure Sullivan
algebras, introduced originally by Koszul in [102]. If (AQ <8> AP,d) is a pure
Sullivan algebra with Q = Qeven and ? = Podd then its Koszul-Poincare series
is the formal series U(z) given by
oo
U(z) = S^ \rzr , ?? = ^(-l)fc dim(AQ ® AkP)r~k .
Lemma 32.11 If 2ai,..., 2aq are the degrees of a basis (yj) of Q and if
2b\ — 1,..., 2bp — 1 are the degrees of a basis (xt·) of ? then
? (i-*26')
U(z) = ?? .
? (?-*2?0
The Rational Dichotomy 445
proof: Write AQ <g> ?? = AV. Clearly U = UAV does not depend on the
differential, and ?Av®aiv = ^av 'U.\w- Since AV = ??/? (8) - · · (8) Ayq q$ ??? <g>
• - · <g> ??? and since (trivially)
Uhyi (z) = _ z2a. and UAxi (z) = l- z2bi ,
the lemma follows. ?
Now while the Koszul-Poincare series U(z) = ]T Xrzr for (AQ&AP, d) does not
?
depend on the differential, it nonetheless gives important numerical information
about H(AQ <g> AP,d). Indeed, since elements of AQ <g> AkP have odd (even)
degree if k is odd (even), ?? is just the Euler-Poincare characteristic of Cr =
?(?<2 <8> AkP)r~k. Moreover, because d decreases wordlength in ? by 1 and
k
increases degree by 1 it follows that d preserves each Cr. Thus ?? is also the
Euler-Poincare characteristic of H(Cr,d):
where Hk{AQ ? ??) is as denned in §32(a).
Now suppose H(AQ <g> AP, d) is finite dimensional. Then H(Cr) = 0 (and
hence ?? = 0) for sufficiently large r; i.e., U(z) is a polynomial. Moreover, since
AQ <g> AP = 0Cr, the Euler-Poincare characteristic ? of H(AQ <g> AP, d) is given
by
x = ? xmc) = ? ?' = w(!) - C212)
proof of Proposition 32.10: Recall the odd spectral sequence (Ei,di) (§32(b))
for (AV,d), whose i?i-term is just the cohomology H(AV,da) of the associated
pure Sullivan algebra. Since this is finite dimensional (Proposition 32.4) and
since Ei+\ = H(Ei,di) the Euler-Poincare characteristics satisfy
??(??,??) = ??? = Xe2 = ¦¦¦ -
Since ??'"* = AFeven <g> AkVodd it vanishes unless 0 < k < dimV01111 and so
Em = Ei, i > diml/odd. But ?,? is the associated bigraded vector space for
H(AV, d) and we conclude that
XH(AV,d) =XH(\v,d„) ¦ C2.13)
On the other hand, if 2??,.... 2aq are the degrees of a basis for yeven and if
2by - 1,..., 2bp - 1 are the degrees for a basis of Vodd then Lemma 32.11 asserts
that the Koszul-Poincare series U for (AV, ??) satisfies
446 32 Elliptic spaces
Since U{z) is a polynomial and since ? = 1 occurs as a root with multiplic-
ity 1 in 1 — zm it follows that ? > q and that ? = 1 occurs as a root of
U(z) with multiplicity ? — q. Since ? = dim V01" and ? = dimVeven we have
shown that dimFodd > dimVeven (another proof is given in Theorem 32.6),
that XH(AV,d) = XH(AV,d„) = W(l) = 0 if dimVodd > dimVeven and that if
dim Vodd = dim Veven = q then
XH(AV,d) = W(l) = ? bj H a,- > 0 C2.14)
This proves the first assertion of the Proposition and the equivalence (i) <=>
(v).
Now clearly (iii) =? (ii) =? (i), and Proposition 32.3 asserts that (iv) =>
(iii). It remains to show that (i) => (iv). If (i) holds then, since (i) => (v),
dimVeven = dim Vodd. Since H(AV,dc) is finite dimensional (Proposition 32.4),
Proposition 32.2(ii) asserts that H+(AV,da) = 0. It follows (Proposition 32.3)
that if (??) is a basis of Vodd then (daX{) is a regular sequence in AVeven and
that H(AV,da) = H0{AV,da) = AV^en/{daxu... ,daxq).
In particular, H(AV, da) is evenly graded and so the differentials dx, d2,. ¦ ¦ in
the odd spectral sequence all vanish. In other words H(AV,da) is the bigraded
vector space associated with H(AV, d). Let y\,..., yq be a basis for yeven. Since
dayi = 0 there are elements ?? e Ayeven <8> A+Vodd such that d(yi + ?^) = 0.
Put y[ = yi + ?? and note that (AV,d) = A{y[,...,y'q)® AVodd. Thus without
loss of generality we may assume d — 0 in Veven.
Finally let xx,... ,xq be a basis of Vodd such that degzi < · · · < dega;, and
suppose by induction that for i < k we have found elements ?? 6 AVeven <g>
j\>3yodd such tfrat ?(?? + ?{) = daX{. Replace x-x by x-t + *i to reduce to
the case dxi = daxu i < k. Then d = da in Ayeven (g) ?(??,... ,xk_i). Since
????,... ,daXk~i is a regular sequence, Proposition 32.3 implies that daxk —
dxk is a c^-coboundary in AVeven <g> A+Vodd and hence it has the form da^k,
some ? a: G AVeven <8> A^3yodd. By construction d^k = da^>k, and so ^?* =
Now set x[ =xi + ^i. Then (AF,d) = A(y[,... ,y'q,x\,... ,x'q), with dj/^ = 0
and dx\,..., cte'g a regular sequence in A(yi,..., y'q). D
Corollary // dim Veven = dim yodd = q then
? A-^)
^2dimHr(AV,d)zr = ?
?2
? / ?
and XH(AV,d) = dimH{AV,d) = ? h ?
i=i I j=\
The Rational Dichotomy 447
proof: Since H(AV,d) *? H(AV,da) = H0(AV,dc) we have in the Koszul-
Poincare series U for (AV, da) that
Ar = ??-1)* dimHk~k(AV>d*) = dimHr(AV,d) .
k
Apply Lemma 32.11. G
(e) Rationally elliptic topological spaces.
Let X be a simply connected topological space with finite dimensional ra-
tional homology. The formal dimension ?? of ?' is the maximum integer
such that ??? (X; Q) ? 0, and its Euler-Poincare characteristic is the integer
Xx = ?{-1)???????(?;<0?). Recall that X is rationally elliptic if the graded
?
vector space ?*(?) <8> Q is also finite dimensional. In this case we set
??(?) = dmiTTevenPO ® Q - dim7roddP0 <g> Q ,
and we let 2??,...,2?? and 2bi — 1,... ,2bp - 1 be the degrees of a basis of
?»(X) (8) <Q>, so that ? = dim 7TOdd(X) ® Q and ? = dim ????? (X) ® Q.
Since the minimal Sullivan model, (AF, d), for X satisfies
, d) = ?*{X; Q) , F = ??. (X) ® Q and cat(AV, d) = cat0 X
we may translate Theorem 32.6 and Proposition 32.10 and their corollaries as
follows:
Theorem 32.15 If X is a rationally elliptic space then, in the notation above,
(i) ??
(ii) ??>? 2aj.
(Hi) 2nx-l>
?=?
(iv) dim7reven(X) ® Q < dim7rOdd(-Y) ® Q < cat0 X-
In particular ?»(X)<g>Q is concentrated in degrees < 2?? — 1 and dim tt»(X)®Q <
Proposition 32.16 If X is a rationally elliptic space then in the notation
above
XX > 0 and X7r(X) < 0 .
Moreover, the following conditions are equivalent:
448 32 Elliptic spaces
(i) Xx > 0-
(ii) H*(X;Q) is the quotient of a polynomial algebra in q variables of even
degree by an ideal generated by a regular sequence of length q.
(Hi) dim 7reven(X) <g> Q = dim ????(?) <g> Q.
// these conditions hold then
)
^2dimHk(X;Q)zk = -± and Xx = -j
k ? (?-*2*') ?
Example 1 Simply connected finite ?-spaces are rationally elliptic.
If G is as in the title then its Sullivan model is an exterior algebra on a graded
vector space Pg of finite dimension concentrated in odd degrees, and has zero
differential (Example 3, §12(a)). The dimension of Pq is called the rank of G.O
Example 2 Simply connected compact homogeneous spaces GjK are rationally
elliptic.
Proposition 15.16 asserts that these spaces have a Sullivan model of the form
{AVbk ® APG,d), where d = 0 in AVbk, Vbk *s concentrated in even degrees,
d{PG) C AVbk and Pg and Vbk are finite dimensional. Note that this is a pure
Sullivan algebra.
In this example we have ??(?/?) = dimV?K — dim Pc = dim ?? — dim Pc,
(even though the Sullivan algebra may not be minimal). Thus
= rank(K) - rank(G) .
The Rational Dichotomy 449
Example 3 (Allday-Halperin [3]) Free torus actions.
Suppose an r-torus ? = S1 ? · · · ? S1 (r factors) acts smoothly and freely on a
simply connected compact smooth manifold M. Then the projection M —> M/T
onto the orbit space is a smooth principal bundle. Hence there is a classifying
map M/T —> BT whose homotopy fibre is homotopy equivalent to M (§2(e)).
Now assume M is rationally elliptic. Since BT = CP°° ? · · · ? CP°° its
homotopy groups are concentrated in degree 2, and since M/T is compact its
homology is finite dimensional. Thus M/T is rationally elliptic. It is immediate
from the long exact homotopy sequence that
??(?/?) = ??(?) + ????) = ??{?) + r .
On the other hand, Theorem 32.15 asserts that ??(?/?) < 0 and so we conclude
that
r < -XM ;
i-e., — Xm is an upper bound for the dimension of a free torus acting on M.
In the case of homogeneous spaces G/K (Example 2, above) this shows that
rank G — rank ? is an upper bound for the dimension of a torus acting freely on
G/K. a
(f) Decomposability of the loop spaces of rationally elliptic spaces.
Let X be a simply connected CW complex. Recall the localization X-p (§9(b))
at a set of primes V, obtained by 'inverting the primes not in V.' Here we prove
Theorem 32.17 (McGibbon-Wilkerson [117]) If the integral homology H.{X\ Z)
is ? finitely generated abelian group and if X is rationally elliptic then invert-
ing some finite set of primes gives a localization X-p such that there is a weak
homotopy equivalence
1—? oh· ? ?—? /?'2?. ¦ 1
Corollary If X is a rationally elliptic finite CW complex then H*(UX; Z) has
p-torsion for only finitely many primes p.
Remark By contrast Anick [9] and Avramov [15] construct simply connected
finite CW complexes X such that Hm(UX; Z) has torsion of all orders.
proof of Theorem 32.7: In the course of the proof we shall use a little 'inte-
gral' homotopy theory not derived in this monograph.
The proof is by induction on dim7r»(X)<8>Q and for purposes of the induction
we prove the theorem under the slightly more general hypothesis that for some
finite set of primes Pi,-..,pn, H*(X;R) is a finitely generated module over
R = ? (±,..., j- J cQ. In this case we may replace X by a CW-p-complex
450 32 Elliptic spaces
(V = primes of R) with cells in finitely many dimensions. In the proof we shall
often extend R (and further localize X) by inverting finitely many additional
primes, and then continue to denote the result by R (and by X). We shall also
denote by Sk the localization 5^,.
We shall frequently rely on the following observation. Suppose given continu-
ous maps X —> ? <^— ? in which g is a rational homotopy equivalence and each
Hk(Y;Z) and Hk(Z;Z) are finitely generated A-modules. Then after inverting
finitely many more primes we can find localizations X-p· —^ Yp· ^— Z-p· and
a map h : X-p· —> Z-p· such that g-p* h ~ f-p<. Indeed, we may assume Y and ?
are CW-p-complexes of finite type and that / maps X into a finite sub CW-p-
complex of Y. Invert finitely many primes to make g a homology isomorphism
between large skeleta and then compose h with the homotopy inverse-
Note as well that by inverting finitely many primes we may arrange that X is
(r— l)-connected and that HT(X\ Z) is a non-zero free i2-module. We distinguish
two cases:
Case 1: r is odd.
Since Hr{X; Z) is a non-zero free A-module there are continuous maps Sr -A-
X -^ K(R,r) such that Tir(gf) is an isomorphism (Theorem 4.19 and Propo-
sition 4.20). Since r is odd the composite is a rational homotopy equivalence
(Example 3, §15(b)). Thus by inverting finitely more primes we can construct
h : X —-> Sr such that hf ~ id.
Let i : F —> X be the 'inclusion' of the homotopy fibre of h. Then (cf. §2(c))
there is a principal fiSr-fibration ?5? —> F —> X. Since ([2]) ??»(?5?,· R) is the
polynomial algebra R[ar-\) and H*(X;R) is finitely generated, a Serre spectral
sequence argument shows that H*(F;R) is noetherian over R[ar~i] and hence
has torsion at only finitely many primes. By inverting these we can arrange that
H*(F;R) is torsion free. On the other hand the Mapping theorem 28.6 asserts
that cato F < oo, and hence (Proposition 32.4) F is rationally elliptic. Thus
H*(F;Q) is finite dimensional and H*(F;R) is a finitely generated A-module.
But dim^(F) ® <Q> < dim7r»(X) <g> <Q> and so the theorem holds for F by
induction. Since fli · ?/ : ?.? ? ?5? —> UX is a weak homotopy equivalence,
the theorem holds for X as well.
Case 2: r is even, r — 2q.
As in the previous case we have Sr —> X -^ K(R, r) with nr(gf) an isomor-
phism. Recall the definition of the special unitary group SU(q). As in Example 3,
§15(f) there is an action of SU(q) on 52?-1 and this gives an associated fibre
bundle ? : ESU{q) xSU(g) S2^1 —»· BSU{q) with fibre S2* (§2(e)).
The calculations referred to in Example 3, §15(f) show that BSU(q)<Q ~
q
Y\ KBi — 1,Q) and that the connecting homomorphism ?» for ? maps a ba-
sis element for K2qBSU(q)Q to 5q9-1. Thus by inverting finitely many primes
we obtain from g : X —> K(R,r) a map h : X —> BSU(q)-p such that
The Rational Dichotomy 451
d,[hf] = [S2"-1].
Use h to pull the spherical fibration back to a fibration S2' -^ ? —>
X whose connecting homomorphism maps [/] to [S2']. This implies that
dim ?» (E) <g> Q < dim ?» (X) <g> Q while a Serre spectral sequence argument shows
that if» (E; R) is a finitely generated A-module. Thus by induction, the theorem
holds for E.
Finally, as in §2(c) we have a fibration ?? —> ?? —> S2' which, after
finitely many primes are inverted, admits a cross-section. Thus multiplication
in CiX defines a weak homotopy equivalence ?? ? S2q~1 -^ ???. ?
Exercises
1. Determine all the elliptic spaces X satisfying dimL^ < 3.
2. Let X be an elliptic space and denote by ? the maximal length of a nonzero
Whitehead bracket. Prove that cato^ > n/2.
33 Growth of Rational Homotopy Groups
In this section the ground ring h is an arbitrary field of characteristic zero.
Suppose that X is a simply connected topological space with rational homology
of finite type. In this section we describe the implications for ?» (X) ® Q of the
hypothesis that X has finite rational category. If dim ?,(?) <g>Q is finite then X
is rationally elliptic (Proposition 32.4) and its properties are described in §32.
Thus the focus here is on spaces X such that dim7r»(X) <g> Q = oo.
Definition A simply connected topological space X with rational homology of
finite type is called rationally hyperbolic if cato X < oo and dim7r»(A'')<g>(Q) = oo.
The justification for the terminology lies in our first main result:
k
• If X is rationally hyperbolic then ? diinKi(X) <8>Q grows exponentially in
i=2
k.
Note that this defines a dichotomy:
• Simply connected spaces X with rational homology of finite type and finite
rational category are either:
- rationally elliptic, with ?*(?) <8> Q finite dimensional, or else
- rationally hyperbolic, with ?,(?) <g) Q growing exponentially.
The result above leaves open the possibility of large intervals [r, s] such that
Ki(X) <8> Q = 0, r < i < s. However, this cannot happen: it follows from
Theorem 31.16 that
• If X is rationally hyperbolic then there are integers ? and d such that
Q^O , k>0.
This assertion can be considerably improved in the case that H*(X; Q) has finite
dimension. In this case we let ?? be the formal dimension of ?; ? ? is the largest
integer such that Hnx (X; Q) ^ 0. Then we show that
• If X has finite dimensional rational homology and formal dimension ??
then either
- X is rationally elliptic and iTi(X) <8> Q = 0, i > 2??, or else
- X is rationally hyperbolic and for each k > 1, Ki{X) <8> Q ? 0, some
i G {k,k + ??).
In particular, if X is rationally hyperbolic the non-zero rational homotopy groups
of X occur at intervals of at most ? ? — 1.
This section is organized as follows:
(a) Exponential growth of rational homotopy groups.
The Rational Dichotomy 453
(b) Spaces whose rational homology is finite dimensional.
(c) Loop space homology.
(a) Exponential growth of rational homotopy groups.
Proposition 33.1 Let X be a simply connected topological space with rational
homology of finite type. If X is rationally hyperbolic then
(i) dim????{?) <g> <Q> = oo.
(ii) For some integers ? and d: iIN+kd{X) ® Q ? 0, k > 0.
proof: Let {AV, d) be a minimal Sullivan model for A'. Then cat(AV,d) =
cato A < oo. For any ? > 2,.divide by V<n to obtain a quotient Sullivan al-
gebra (AV-n,d), which also has finite category (Mapping theorem 29.5). Since
dim7Tp(A") ® <Q> = dim Vp, ? > 1, it follows that V~n ? 0. If V-n were concen-
trated in even degrees we would have d = 0 and H(AV-n,d) = AV-n would be
a polynomial algebra, contradicting cat(AV-n,d) < oo. It follows that Vodd is
infinite dimensional, which proves (i).
Let L be the homotopy Lie algebra of (AV, d). Since Lk is dual to Vk+1, Leven
is infinite dimensional. Thus according to Theorem 31.17 for some non-zero
? G Leven and some ? G L the iterated Lie brackets (ad a)k? are all non-zero.
This proves (ii). ?
Theorem 33.2 Let X be a simply connected topological space with rational
homology of finite type. If X is rational hyperbolic then for some C > 1 and
some positive integer K:
k
^2 dim ???(?) ® Q > Ck , k> ? .
?=0
proof: Let (AV,d) be a minimal Sullivan model for X. As in the proof of
Proposition 33.1 we have to show that because cat(AF, d) < oo and dim V = oo,
? dim V1 > Ck, k > ? for suitable C and K. Set m = cat(AV, d).
Now for any integer k dividing by V<k gives a quotient minimal Sullivan alge-
bra which we can write as (AVffc·2*-2! ® AV^2*, d), where V^ = {Vl}k<i<r
By minimality d — 0 in l/[*>'2*-2]. Moreover, this quotient Sullivan algebra has
category < m (Mapping theorem 29.5) and hence the product of m +1 cohomol-
ogy classes is always zero (Corollary to Proposition 29.3). Thus in particular,
454 33 Growth of Rational Homotopy Groups
Define ? : V^2* —» AFt*·2*) by requiring that d = a + ? with Im/3 in
the ideal generated by V^2*-1. If ?? is the component of ? in ArVl*'2*~2] then
we have
m+l
Am+lVr[*,2*-2] = ? up (V5:2*-Ij .Am+
Since A^'*'2*' is concentrated in degrees i e \pk,2pk - 2p] it follows that
2k-2
Now for each integer k set A(fc) = ^ dim V*. Denote 2m+1 (m + l)!m by c
i=k
and use Proposition 33.1 (ii) to find an integer JV such that
\(k) >c3m , k>N.
Then it follows from the inequality above that if k > N,
v ' *->' p=2
This in turn implies that for some ? G [2, m + 1],
In particular, \{pk - 1) > A(fc) and \(pk - lI^-1 >
L J
Iterating this process yields a sequence of integers No < Ni < ¦ ¦ ¦ such that
No = ? and 7Vi+i = piiVj - 1 (some pt G [2, m + 1]), and such that
L cm J
But since Ni+1 > |^ we have ? ? < i g (§)* = &. Thus cS ^ <
0 ' i-0
and it follows that
Now for any i > 1 and for Ni <r < Ni+i we have r < (m 4- lJ7Vi_i and
The Rational Dichotomy 455
In other words, setting C - \^?\ (m+1Jjv we have ? dim Vn > Cr, r > ?. ?
L Ct" J n=0
(b) Spaces whose rational homology is finite dimensional.
Theorem 33.3 Suppose X is a simply connected topological space with finite
dimensional rational homology and formal dimension ??. Then either tti(X) ®
Q = 0, i > 2nx or else for each k > 1, ??(?) ® Q ? 0 for some i G (k,k + nx).
proof: If dim n*(X)®Q is finite then Theorem 32.15 asserts that Ki(X)®Q = 0,
i > 2??. Thus if X were a counterexample to the theorem there would be
integers k > 1 and ? > ? ? + k such that ?{(?) ® Q = 0, k < i < i, and
ne(X) <8> Q is non-zero. It follows (Theorem 15.11) that a minimal Sullivan
model for X (over any field Jk of characteristic zero) has the form (AU <g> AW, d)
in which U = U-k and W = W-* are non-zero graded vector spaces and We ? 0.
Moreover, H^AU<g> AW,d) ^ H^X; jfe) = 0, i > ??. We shall show that this is
impossible.
Denote by (AW, d) the quotient Sullivan algebra and let b be a variable of
arbitrary degree. The main step in the proof is
Lemma 33.4 In the situation of the proof of Theorem 33.3 suppose given a
morphism of the form g : (AU,d) —> (??,?). Then the identity in Ab extends to
an isomorphism of cochain algebras
Ab ®au (AU ? AW, d) = (Ab, 0) ® (AW, d) .
proof: Given any Sullivan algebra (AV, d), define V by Vk = Vk~1 and denote
corresponding elements by ? <—> ?. Define a commutative cochain algebra
(AV ®(Jc®V),S\ as follows:
• Multiplication by elements in AV is just multiplication on the left in AV <g>
V), and V-V = 0.
• ? = d + s, where s is the derivation defined by sv = ? and sv = 0, and
where ?(? <g> 1) = ?? <g> 1 and dv = -sdv.
This construction is natural: if ? : (AV, d) —> (AZ,d) is a morphism of Sullivan
algebras, extend it to ? : (AV ®(lk®V),s) —-> (?? ® (? ® ?), ?\ by setting
?? = sipv. Moreover, division by V defines a natural surjective cochain algebra
morphism ? : (AV ®(&®?),?) —> (AV, d).
In particular, denote the cochain algebra (AC/ ® (k ? U), ? ) simply by (?, ?).
The key observation is then that there is a relative Sullivan algebra
456 33 Growth of Rational Homotopy Groups
such that applying AU ®a — yields the original relative Sullivan algebra AU —>
AU ® AW. The derivations d in .4 and in AU ® AW extend uniquely to a
derivation d in A® AW = A® au (AU ® AW). Now extend the derivation s in .4
to a derivation s in A ® AW by constructing its restriction to W as a linear map
W —> AU ® U ® AW such that dsw = -sdw, w ? W. Then sd + ds = 0, and
s2 = 0 because s will automatically vanish in AU ® C/ <8> AW. Thus J = d + s is
a differential.
Suppose by induction that s is defined in W<r. If w ? WT, then by construc-
tion dsdw = —sd2w = 0, and so sdw is a d-cocycle in AU ® C/ <8> AW. Since
r > ? > k + ?? it follows that degsdw > k + ?? + 2. On the other hand, U is
concentrated in degrees <k + l and H(AU ® AW, ci) is concentrated in degrees
< ??. Hence //'(AC/ <8> f/ <g> AW, ci) = 0, ?" > k + nx + 2, and sdw must be a
d-coboundary. Thus we may extend s to WT so dsiu = —sdw, w ? Wr. This
constructs ?.
Next, given ? : (AC/, d) —-> (Ab, 0) extend it to ? : (?, ?) —+ [Ab ® (Jk © ieb),s\
as described above. Define the quotient cochain algebra (?,?) by
IAb ® (Jk ® Jkb), degoiseven.
?6 ® (ic ? 3cb)/bb, deg ? is odd.
Set E ® AW,5) = 5 ®? (? <8> AW,5). By inspection H(B,8) = A, and
so the quotient map ? : (B ® AW, ?) —> (AW, d) is a quasi isomorphism
(Lemma 14.2). Choose a morphism ? : (AW,d) —> (B ® AW, ?) such that
¦?? = id (Lifting lemma 12.4); then multiplication defines an isomorphism
id-? : (?,?) <g> (AW,d) -=» (B <S> AW,?), as follows by filtering using the de-
gree of B.
Finally, dividing by b gives a morphism (?,?) —> (Ab,0) and
(?6 ???/ (AC/ <8> AW, d) ^ Ab®BB ®A (A ® AW, ?) s (??, 0) ® (AW, d) . ?
We now return to the proof of Theorem 33.3, where we show that
• Both ? (AW, d) and H(AU,d) are finite dimensional. C3.5)
Suppose C3.5) is proved. Filter AU® AW by setting FP(AU®AW) = (AU)-P®
AW and observe that the ?2-term of the corresponding cohomology spectral
sequence is given by E%'Q = Hp (AU ,d)® Hq (AW,d). If ? and q are the maximum
degrees in which H(AU,d) and H(AW,d) are non-zero then for obvious degree
reasons d,- = 0 in Ef'\ i > 2 and E?'9nlmd; = 0, i >2. It follows that ?™ ? 0
and hence that HP+Q(AU <g>AW,d) ? 0. But (AW,d) is a non-trivial minimal
Sullivan model and W = W-e with ? > k + nx > ??. This contradicts the
hypothesis that HX(AU ® AW, d) = 0, i > ? ?, and establishes the theorem.
Now we have to prove C3.5). Fix a basis u\,... ,ur of U such that degtti <
deg u-2 < · · · and let (AC/; ® AW, Di) be the quotient Sullivan algebra obtained by
The Rational Dichotomy 457
dividing by m,..., Uj_i. We show first by induction that each H (AUi ® AW, A)
is finite dimensional so that, in particular, H(AW,d) is finite dimensional.
Indeed suppose H(AUi<S>AW, Di) is finite dimensional. If degu* is even extend
this to the Sullivan algebra (AUi ® AW ® Ab, Di) by setting ?? = ui; and
note that this is quasi-isomorphic to (AC/j+i <g> AW,Di+1). Thus the long exact
cohomology sequence associated with
0 —»· (AUi®AW,Di) —»· (AUi®AW®Ab,Di) —»· (?[/;®??··', ?><) ® (Jfco, 0) —»· 0
shows that //(At/j+i ®AW,Di+i) is finite dimensional.
On the other hand, if deguj is odd write ?>*(]. <8>?) = l®.Dj+i<i> + Uj<g>o<i>. ? G
AC/j+i <g>AW. Let ? be the maximum degree such that HN (AUi® AW, DJ ? 0.
If ? is a .Dj+i-cocycle representing [?] ? ? (AUi+i® AW, Di+i) and ifdeg? > ?
then U{ ® ? is a .Dj-cocycle and hence of the form Dj(uj <g> ? +1 <8> ??). It follows
that .Dj+??? = 0 and ?(?)[??] = [?]. This procedure constructs an infinite
sequence [?] «— ?(?)[??] 4 .
But division by (uj), j ? i defines a surjection (AC/, d) —> (Auj,0). According
to Lemma 33.4, Auj ®AU (AU ® AW,d) ^ (Aui,0) ® (APF, J). It is immediate
that if xi,... ,xr is the dual basis of the homotopy Lie algebra L of (AU,d)
then the holonomy representation hi' of L in H(AW, d) satisfies hl'(xi) = 0
(Introduction to §31). Since L is finite dimensional adz,- is trivially nilpotent.
Hence (Lemma 31.9) ?(?) is locally conilpotent and [?] = 0; i.e. H>N(AUi+l ®
AW,Di+i) = 0. This shows that H(AUi+l ® AW,Di+i) is finite dimensional.
It remains to show that H(AU,d) is finite dimensional and for this we lose no
generality in assuming Jk algebraically closed. Consider an arbitrary morphism
? : (AU,d) —> (??,?) where dego = 2. It extends to a morphism ? : (AU ®
AW, d) —> (Ab,O) defined as follows: use Lemma 33.4 to identify Ab®au (AU ®
AH7, d) as (??,?) <g> (AW,d) and let ? be the composite
(AU ® AW, d) —»· Ab ®au (AU ® AW, d) —»· (?6,0) .
Suppose now by induction that ??,\ = ¦ ¦ ¦ = nui-i = 0. If deguj is odd then
nui = 0. Otherwise factor ? to give a morphism ? : (AUi ® AW, Di) —> Ab.
Since ? (AUi ® AW,Di) is finite dimensional and since D{Ui = 0, it follows that
uf = Dfi, some N. Hence 7r(uf ) = n(uf) = W(Diu) = 0; and it follows that
¦nui = 0. Thus ? = 0 in U and the only morphism (AU, d) —> (Ab,0) is the
trivial one. Now Proposition 32.3 asserts that H(AU,d) is finite dimensional.
This completes the proof of C2.5) and hence of the theorem.
D
Proposition 33.6 (Lambrechts [104]) Suppose X is as in Theorem 33.3. Then
for r sufficiently large,
d\mH*(X;<
;=r+i v '
458 33 Growth of Rational Homotopy Groups
proof: Let (AV, d) be the minimal Sullivan model of X and set
? = dimiT(X;Q) = dim H {AV, d).
If the inequality of the Proposition fails for some r > nx +1 we show by induction
that for 0 < k < ?? — 1, (AV, d) can be decomposed as a relative Sullivan algebra
of the form (AUk®AWk,dk) in which Wk = (Wk)-r, (WkY = 0, r+1 < i < r + k
and
?+??—1
Y^ ? dim Wi < dim Wk .
Since Vk = irk(X) ® Q, this can be achieved for k = 0 by setting Uk = V<r.
For the inductive step, let w ? (Wk)r+k+l and write dw = aw + ?w with aw ?
) ® W? and ?w ? AUk. Since cPw = 0 it follows that aw ? kerd® W?. Let
k1
H (a) : (Wk)r+k+1 —> ffiAV)®^ be the induced map and write ???? = ?® ?'
where ? is the smallest subspace such that lmH(a) C H(AV) ® Z. Then by
replacing a basis Wi of Wr+/:+1 by elements of the form w[ = Wi + ?{ with
?,· ? AUk ® Z' we may arrange that ? : Wr+k+1 —> AC/* ® (Jfe ? ?). Set
C/fc+i = Uk ? ? ® (Wk)r+k+1 and set VKfc+1 = ?' ® Wj%+k+2. Since (clearly)
iVdim(Wfc)r+/:+1 > dim ? it follows that ' ? ? dimW* < dim ?'. This
i=r+k+2
closes the induction.
For k = ?? — 1 we thus obtain a decomposition (AV, d) = (AU <g> AVF, d) in
which W = W-r, Wr ? 0 and Wl = 0, r + 1 < i < nx + r - 1. Extend a non-
zero linear function / : H/r —> ?c to a derivation 0 in (AC/ <g> AWr, d) by putting
O = 0 in U. Suppose ? is further extended to (AU <g> AW^m,d). \i w ? Wm+l
then 6dw is a cocycle of degree at least ?? + 1, and hence is a coboundary in
AV. Thus we can extend ? to Wm+1 so that Odw = duw, w € Wm+l.
This exhibits / : Wr —> Ik as a non-zero Gottlieb element (§29(d)) for (AV, d).
Since cat(AVr, d) is finite there are such elements in only finitely many degrees
r (Proposition 29.8 (ii)), and hence the inequality of this Proposition holds for
sufficiently large r. ?
(c) Loop space homology.
For any topological space X with rational homology of finite type, the Poincare
oo
series for X is the formal power series ?? = X)dimfin(X;Q)zn. In particular,
?
if X is simply connected the Milnor-Moore theorem 21.5 states that the loop
space homology of X is isomorphic to the universal enveloping algebra of the
homotopy Lie algebra Lx : H*(UX;Q) = ULx. Denote by ? (or by r{(X)
when the dependence on X is not clear from the context) the integers
?? = dim ?» (?.?) <g> Q =
Then the Poincare-Birkoff-Witt theorem 21.1 identifies Pqx as the formal power
The Rational Dichotomy 459
?(? + *2?+1?+1
??? = ^ - C3.7)
(Compare with the formula in §32(d) for ?? for certain rationally elliptic spaces!)
Note that C3.7) provides algorithms for computing the integers dim Hi(flX; Q).
1 < i < ? from the integers dim7Tj+i(X) ® Q, 1 < i < ? and for computing
the integers dim7ri+i(X) ® Q, 1 < i < ? from the integers dimHi(ttX;Q),
1 < i < N.
Proposition 33.8 Suppose fT(X;Q) = 0, i > nx. Then the integers
dimHi(ClX;Q), 1 < t < 3(?,? — 1) determine whether X is rationally elliptic or
rationally hyperbolic.
proof: Theorem 33.3 asserts that X is rationally elliptic if and only if ttj (X) <g>
Q = 0, 2?? < j < 3?? — 1. This only requires the calculation of ru 2?.? — 1 <
i < 3nx - 3. D
Proposition 33.9
(i) If X is rationally elliptic then there are constants 0 < A < ? such that
?
??? < ? dim Ht(IIX; Q) < Bnr , ? > 1 ,
where r =
(ii) If X is rationally hyperbolic and if Hl(X;Q) = 0, i > ?? then there are
constants C > 1 and ? such that
k+2(nx-l)
? dimHi{nX;Q) > Ck , k>K.
i=k+l
proof: The first assertion is immediate from C3.7). For the second let (AV, d)
be the minimal Sullivan model of X. An element ? of V of least degree is a
cocycle of degree ? < ??. If ? is even then vp is a coboundary for some ? such
that (p — l)n < ??. Thus whether ? is even or odd it follows that V has a
non-zero element of odd degree 2k + 1 < 2?? — 1, and so Lx has an element
00
of even degree 2k < 2?? - 2. By C3.7), ??? - 1Jzak ? riz* is a power series
i=2
with positive coefficients. Now apply Theorem 33.2. ?
The radius of convergence R of a power series Y^anzn is the least upper
bound of the nonnegative numbers r such that X) |an|rn converges, and is given
460 33 Growth of Rational Homotopy Groups
by A = limsup lunl1/". Recall that X is supposed simply connected with
rational homology of finite type.
Proposition 33.10 The formal power series Pqx and ? rnzn have the same
radius of convergence, R. Moreover
(i) R = 1 if X is rationally elliptic and R < 1 if X is rationally hyperbolic.
(ii) If X is rationally hyperbolic and if ??(?;^ = 0, { > ?? then R < ? < 1
for some constant ? depending only on ??.
proof: Write Y^anzn <C Y^bnzn if an < bn for all n. Since
it follows by C3.7) that X)rn2n is convergent if and only if Pqx is convergent.
If X is rationally elliptic the products in C3.7) are finite and R = 1. If X
is rationally hyperbolic let cato X = m. The proof of Theorem 33.2 shows that
given ? sufficiently large there is an infinite sequence of integers Ni such that
? = No, Ni < Ni+i <(m + l)iV; and
/2N-2
j=N
J
where cm is a constant depending only on m. It is also shown that for ? suffi-
ciently large the right hand side is larger than one. It follows that lim sup r\[n >
1. Moreover, Theorem 33.3 states that if i/l'(X;Q) = 0, i > nx, then when
? = 2n.\;e3x the right hand side is at least 21/N, from which assertion (ii)
follows at once. ?
Example 1 Wedges of spheres-
Suppose X = V 5n>+1 with rii > 1 and at most finitely many spheres of a fixed
i
degree. Then the homotopy Lie algebra is a free Lie algebra Ly on a graded
vector space with basis (v{) and degUj = ni (Example 1, §24(e)). But ULv is
the tensor algebra TV (§21(c)) and thus
?-?>?;
i
Example 2 X = S3 V S3.
The Rational Dichotomy 461
As in Example 1, PQX = ^^ and hence Ht(UX;Q) is concentrated in even
degrees. Thus formula C3.7) becomes
l-2z2
where r2n = dim7r2nQ(S3 V S3) <8> Q- Take logs of both sides to obtain
y2nk
??
?
k ? k
Equating the coefficients of z2N gives J^ v^da = 2N.
d\N
The Mobius function ?(?) is defined by: ?(?) = 1, (—l)r or 0 as ? is 1,
a product of r distinct primes or divisible by a prime squared. Elementary
number theory gives
as a precise formula for the dimension of ?2^?(?-?) ® Q· As observed above,
) 0. D
Example 3 X = S? V Sf U[a[a<?]w]w D8.
Here ? and ? are the elements of ?3 (S? V Sf) represented by Sf and S|,
and [—,— ]w is the Whitehead product. According to Example 4, §24(f) X
has a Lie model of the form (L,d) = (L(v,w, u),d) with degu = degu; = 2,
deg u = 7, du = [?, [?, w]} and dv = dw = 0. In degrees < 8 the cochain
algebra C*(L,d) coincides with (A(x3,y3, z5,r7, S7,as),d) where x,y,z,r,s and
a are dual (up to sign) respectively to v, w, [v, w], [w, [w, v]], [v, [v, w]] and u, and
where dz = xy and dr = yz, ds = xz — a and da = 0. Dividing by elements
of degree > 8, and by r and y ? gives a commutative model for X of the form
(A,d) = (A(x,y,z),d)/(yz).
The quotient map q : X —> Sf is represented by the inclusion (Ax, 0) —>
{A,d). Extend this to a quasi-isomorphism from a relative Sullivan algebra,
(Ax <g> AV,d) -=> (A,d). Since {A,d) is Ax-semifree this induces a quasi-
isomorphism k <8>?? (Ax <g> AF, d) -^> Jfe <8>?? (A,d) — Proposition 6.7 (ii). But
-& ®Ax {Ax <8> AV, d) = (AV, d) is a Sullivan model for the homotopy fibre F of q
(Theorem 15.3), while k <g>Al (.4,d) = (A(y,z)/yz,0). This shows that
F=Q53V55.
Let j : F —> X be the map corresponding to the fibre inclusion of the fibration
associated with q : X —> Sf and let i : Sf —> X be the inclusion. Since qi = id,
?? · Clj : USf x ?.? —> ?? is a weak homotopy equivalence. In particular, (cf.
Example 2)
D
462 33 Growth of Rational Homotopy Groups
Example 4 X = T{S3,S3,S3).
Recall that S3 is a CW complex with a 0-cell and a 3-cell; thus the product
S3 x S3 x. S3 has 8 cells consisting of a 0-cell, the three spheres, three 6-cells
attached by Whitehead products and a 9-cell. The 6-skeleton of S3 x S3 x S3 is
called the fat wedge T(S3,S3,S3).
The inclusion q : X —> S3y.S3y. S3 can be represented by ? : (A(x, y,z),0) —>
(A, d) where A is a commutative model concentrated in degrees < 6. It follows
that A = A(x,y,z)/xyz.
If we extend ? to a quasi-isomorphism (A(x,y.z) <8> AV,d) —> (A,d) from a
relative Sullivan algebra then, as in Example 3, the quotient Sullivan algebra
(AV, d) is a Sullivan model for the homotopy fibre F of q. But there are quasi-
isomorphisms (AV, d) ~ (A(x, y,z) <g> AV <8> A(x,y, z),d) = (? ® A(x,y,z),d),
where dx = x, dy = y and dz = z.
Consider the short exact sequence
0 —> Ikxyz ® A(x,y,z) —> A(x,y,z) <g> A(x, y,z) —> A <g> A(x, y,z) —> 0 .
Since the central term is contractible it follows that every cohomology class in
H (A ® A(x, y,z)) is represented by a cocycle in A2(x,y,z) ® A(x,y, z). The
product of two such cocycles is zero and it follows that F is formal and thus
has the rational homotopy type of a wedge of spheres (Proposition 13.12 and
Theorem 24.5). The long exact cohomology sequence also shows that Pp =
A_fT3K ¦ Thus (Example 1)
\-??/{\-?2K A-
Since n*(q) is (trivially) surjective it follows that
1
(l-22K-27 '
It follows in particular that dim7r3(X) ® Q = 3, dim7rg(X) <8> Q = 1 and
that ???(.?) <8> Q = 0, 4 < i < 7. This interval of four consecutive zero rational
homotopy groups is the maximum allowed by Theorem 33.3, since H{(X; Q) — 0,
i > 6. ?
Example 5 X = Y V ?.
Suppose Y and ? are simply connected CW complexes. Let j : F —> ??/ ?
be the 'inclusion' of the homotopy fibre of the inclusion q:Y\l ? —> ? ? ?. The
construction of F (§2(c)) identifies it as F - ?.? ? PZ [j PY ? ??. Since
QYxQZ
the pairs (??,?,?) and (CQ.Y, ?.?) are homotopy equivalent (elementary), and
similarly for ? we have
F ~ QF ? CQZ (J CQF ? ?? = ?? * ?? ~ ?(?? ? ??) ,
QY'xQZ
The Rational Dichotomy 463
(A.15) and Proposition 1.17 — note that (?,?,??) and (?,?,??) are well based
by Step 1 of Proposition 27.9). This shows that F has the rational homotopy
type of a wedge of spheres (Theorem 24.5).
NowsinceF~?(ri:KAQZ),PFB) = z(PqY-1)(Pqz-1). Thus (Example 1)
since ?, (q) is clearly surjective,
and
? - (??? - \){??? - ?) "? ? - (??? - \){??? - ?) ·
Simplifying gives the classical formula
Exercises
1. Let X be a simply connected CW complex of finite type. Suppose that
??(?) <g> Q = 0 for r > n, and HP(X;Q) = 0 for ? < ? < 2n + 1. Prove that
H?{X;Q) =0forp>n.
2. Denote by R the radius of convergence of the series ?)n dimi/n(QX;QJn.
If X is a hyperbolic space prove that for large r and for any ? > 0, we have
where m =
3. Let /?? be the radius of convergence of ?*(??; Q)[z] and P(z) the Poincare
series of H*(X;Q). Suppose that dimH*(X;Q) < oo. Prove that Rx >
min{|2|, ? ? 0 and ? - ? (?) + 1 = 0}.
34 The Hochschild-Serre spectral sequence
In this section the ground ring is an arbitrary field h of characteristic zero.
In this section we fix a graded Lie algebra, L = {L{}ieZ, with universal en-
veloping algebra UL. If M is a left (right) [/L-module then restriction of the
action of UL to L defines a left (right) representation of L in M, and this
correspondence is a bijection (§21(a)). We use this to identify L-modules as
UL-modules and conversely. In particular, the trivial representation of L in ic
defines a canonical (left and right) UL-module structure in Ic.
Now suppose / C L is an ideal and ? is a right L-module. Restriction to /
makes ? into a right /-module, and so the graded vector spaces Ext^/(A, N) are
defined (§20(b)) via any [//-projective resolution of Ik. It turns out (§34(a)) that
the L-module structure in ? makes each Ext?)/(Jc, N) into a right L/7-module.
Thus if M is any right L//-module the graded vector spaces
are defined.
On the other hand, the quotient map L —> L/I makes M into a right L-
module. The main objective of this section is to construct a spectral sequence,
due to Hochschild and Serre [92], which converges from
E%'9 = ExtpUL/I(M,ExtqUI(fc,N)) => Ext?+9(M,jV) .
The case ? = UL will be of particular importance in the applications.
The key notion in this section is that of a chain complex of UL-modules.
Recall from the introduction to §20 that this is a complex of the form
0 <- POf. 4- Plt. 4-
in which each P^» is a (graded) UL-module with (Pi,*)j = Pi,j-i, and d is
homogeneous of bidegree (—1,0). We shall adopt the
Notation convention: A chain complex of C/L-modules will be denoted by P, =
{Pi}, with Pi denoting the UL-module Pit*. If ?? is a UL-module then
Hom?/?,(P*; N) denotes the complex
0 —»· EomUL (Po, ?) ? Homf/L (?, ?) ? - · ·
of graded vector spaces with ? homogeneous of degree 1 (bidegree A,0)).
This section is organized into the following topics
(a) Horn, Ext, tensor and Tor for C/L-modules.
(b) The Hochschild-Serre spectral sequence.
(c) Coefficients in UL.
The Rational Dichotomy 465
(a) Horn, Ext, tensor and Tor for C/L—modules.
Let M and ? be right L-modules. A natural right L-module structure in
Hom(M, N) is then defined by
(f-x)(m) = (-1)*** d^(f(m).x- f(m.x)) , { ? Hom(MJV),
Clearly for any ideal / C L,
?????^?,?) = {/ e Eom(M,N)\f-x = 0,x ? /} .
Thus Hom{//(M, N) is a sub L-module in which / acts trivially; i.e., it is an
L//-module
In particular, let ?, -^ ? be a C/L-projective resolution (§20(a)). The
Corollary to Proposition 21.2 implies that P. is also C/Z-projective, and so
Exti//(M. N) = H (Hom?//(P.. N)). But the remarks above identify Hom?//(P,, iV)
as a complex of L//-modules. which endows H (Homf//(P«,iV)) with a right
L//-module structure. Moreover, given a second such resolution Q* —>· M.
the unique homotopy class of L-linear quasi-isomorphisms Q* -^ P. induc-
ing the identity in M induce L//-linear quasi-isomorphisms Homi//(P«,./V) ^=>
Homi//(Q.,iV). Thus the identification H (RomUI(P,,N)) = H (Homy/iQ.,^))
is an identification of L//-modules and so this endows each Ext?)/(il/, N) with
the structure of right L/7-module.
Definition The L//-module structure above is called the canonical LjI-struc-
ture in Extui(M,N).
Again consider two right L-modules M and N. As with Hom(M, N), the
tensor product M <g> ? inherits a right L-module structure:
(m®n)-x = (-l)degndegIm-x(8)n + m(8)n-x , ? ? L, m ? ?, ? € ? .
As usual, let (M®N) · L denote the subspace spanned by the elements (m<8>n) · x.
Lemma 34.1 If M or ? is UL-free then M <g> ? is UL-free.
proof: Suppose ? is C/L-free on a basis aa. Since M <g> iV = 0 M <g> (?^ · C/L)
it is sufficient to prove that M <g> UL is C/L-free. Let Fp C C/L be the linear span
of elements of the form x^ x;9, ?,„ ? L, q < p. If m G ?/ and a ? Fp then
(m<8>l)-a — m®fl? M <g> Fp_i. It follows that M ® UL is C/L-free on a basis
m\ <8> 1 where m\ is any it-basis of M.
The proof when ?/ is C/L-free is identical. ?
Next observe that a left L-module structure in ? is defined by
x.n = -(-l)degldegnn-x . n?N,x?L.
466 34 The Hochschild-Serre spectral sequence
Moreover, (M ®N) · L is the kernel of the surjection M®N —> M®ULN and of
the surjection M®N —> (M® N) ®ul &¦ This provides a natural identification
M ®UL ? - (M ® N) ®UL h . C4.2)
Furthermore, if / C L is an ideal then (M <g> N) · I is an L-module and so
the quotient ? ®?? ? — (? ® ?) ®ui 3k inherits a natural structure of right
L//-module.
Recall (§3(a)) that we denote Hom(-, Jfc) simply by (-)K
Lemma 34.3 Suppose M and ? are right L-modules and that I c L is an
ideal.
(i) Each Tor^ (M,N) is naturally a right L/I-module.
(ii) There are natural isomorphisms ????(?, ?) S Tor^/(M®iV, k) ofL/I-
modules.
(in) There are natural isomorphisms Ext^/(M, iVs) = Tor^//(M, iV)" of L/I-
modules.
proof: Let P, —> Mbea i/L-projective resolution (§20(a)). It is i//-projective
by the Corollary to Proposition 21.2.
(i) Note that ?????(?, ?) is the homology of the complex P, ®VI ? of right
L/7-modules.
(ii) Note that Pt®N^M®Nisa, C/L-projective resolution (Lemma 34.1)
and hence that TorUI(M®N, k) = ? ((P. ® N) ®VI k). But (?, ® N)®UI& =
P. ®ui N as L/7-modules.
(iii) Write Tor^(M, N)* = {Tor^(M, N)s} and (P. ®ui N)* = {(Pk ®VI iV)«
But clearly Horn (M ®ui N, Je) = Homf//(M, Ns), and since Ik is a field it follows
that
Tor^M, Nf = H ((P. ®ui N)s) = H (Hom?//(P., iVd)) = Ext^(M, iV«) .
Example 1 Tor^^k, k) = s (//[/, /]).
Again let I C L be an ideal. Denote by [/, I] the ideal in L which is the linear
span of the elements [y, z], y,z G /. If ? G / denote by (x) the image of sx in
the suspension s (//[/,/]). We shall establish an isomorphism
Torf7 (A, A) = 5 (//[/,/])
and show that the representation of L/I in Tor^/(ic,i:) corresponds under this
isomorphism to the representation
The Rational Dichotomy 467
In fact, in §22(b) we constructed a Ul-ivee resolution P» (Propositions 22.3
and 22.4) of k explicitly, of the form
k<—UI 4- sI®UI 4- A2 si ®UI 4 .
Apply - <8>ui k and note from the definition that in this quotient d(sx A sy) =
(-l)degsIs[x,y]. Thus
Tor^i*, Jb) = sI/dA2sI = s (//[/, /]) .
Exactly the same way we have a UL-kee resolution Q* of k of the form
Jfc <— t/L A sL®UL^- A2 4
and the inclusion of P« in Q* is a quasi-isomorphism. Let ? G / and y G L.
Then the formula for d in §22(b) gives
d{sx A sy ® 1) = (-l)degSI+degsysx ®y±sy®x + (-l)degsxs[x, y] ® 1 .
Applying ®mk kills sy ® x, while sx <S> y = (sx <S> 1) · y. Thus in homology we
have
(x)-y.= (-i)degi([x,y]) , xel,yeL. ?
Example 2 The representationof L/I in Torj^ (k,k).
As in Example 1 the inclusion of P, in Q* is a quasi-isomorphism of Ul-kee
resolutions of k, and in particular applying — ®ui k gives a quasi-isomorphism
(As/, d) -=> Qt ®ui k. Let a G A9s/ be a cycle (representing a class ? €
Torj^A, A) and let y € L. Then the formulae of §22(b) show that in Q. <S>ui k,
where ? · y is defined by
(SX! ? · · · ? SX,) · y = (_l)deg(sxiA-Asx,) J-> (_l)deg j/ ???(???+??.-??3:,M?? ? . . . ?
s[x,-,y]A ··· ? sx,.
It follows that the representation of L/I in ???1^1 (k, k) is given by
(b) The Hochschild-Serre spectral sequence.
In this topic we construct the spectral sequence. Fix an ideal I C L and a
right L//-module M and a right L-module N. For any graded vector space S
an isomorphism
? : Hom(M <s> S, N) -% Horn (M, HomE, iV))
468 34 The Hochschild-S erre spectral sequence
is given by (pf(m)(s) = f(m <g> s), f G Hom(M <g> S,N).
Lemma 34.4 If S is a right L-module then ? restricts to an isomorphism
EomUL(M ®S,N) A Homw// (M, Hom^S, ?)) .
proof: It is immediate that ? is L-linear. Thus if / G Hom?/?,(M ® 5, iV) we
have f -x = 0, ? e L and so (</>/) ·? = 0. Thus y?/ is L-linear. For ? e I
and m G M it follows that (?/)(??)·? = ?/(??·?) = 0. Thus ?/(??) is /-
linear; i.e. ? : ??????,(? ® 5, ?) —> Hom^// (M, Hom^S, ?*)). Conversely
if / G Hom(M ® 5, iV) and if </?/ G EomUL/I (M, HomujiS, N)) then ?/ is
L-linear and hence so is /. D
Now we construct the spectral sequence. Choose a UL/I-free resolution em '¦
Pt -^> M and a t/L-free resolution ? : Q* —> Jk. Denote the tensor product of
these two complexes by (J2., d) = (P., d) <S> (Q*,d) : Rk = 0 Pp® Qg. (Note
as in §20(a) that each Pp and Qq is itself a graded vector space!) Observe that
• Each Rk is a free UL-module (Lemma 34.1).
• em <S> ? : R» -^> M is a UL-free resolution (because ik is a field).
Now Ext?/L(M, N) is the homology of the complex HomuLIR*, N) and this is
in fact a bigraded complex with
(A.,iV)= 0 Homf/L (Pp ® Qq, N) .
Refer to A; as the total homological degree to distinguish it from the 'normal'
degree inherent in the fact that ? and Rh are each graded vector spaces.
Filter the complex EomUL(Rt, N) by the subspaces 0 Homt/L (Pi <S>Q*,N).
i>p
Use this and the total homological degree to obtain a first quadrant cohomology
spectral sequence, convergent to Ext{/i,(M, N) and with
??'9 = EomUL{Pp®Qq,N) .
Lemma 34.4 identifies HomUL (Pp®Qg,N) = Hom^// (Pp,Hom?//(Q9,iV)).
The differential do is induced from the differential in Qt. Thus because each Pp
is [/L/7-projective we have
E['q =
= Homl/L//(Ppi#«(Homl//(Q*liV)))
= Homi/L//(Pp,Ext9w(l;,iV)) ,
The Rational Dichotomy 469
where Ext^ (A, iV) is equipped with its canonical right L//-module structure.
The differential, di, is then induced from the differential in P., so that
E™ = Ext* L// (M, Ext^A, N)) .
It is immediate that this spectral sequence is independent of the choice of reso-
lutions P, and Qt and so we may make the definition:
The spectral sequence above, converging from Ext^L// (M, Ext^A, N))
to Ext?^9(M, N) is called the Hochschild-Serre spectral sequence.
Remark If / acts trivially in ? then the u^-term of the Hochschild-Serre
spectral sequence may be identified as
where Tor^A, A) has the UL/I structure of Lemma 34.3(i).
In fact in this case
??'" = Homi/L (PP®Qq,N) = HomUL/I(Pp®ULQq,N)
= HomUL/I(Pp <S> (k ®ui Qq),
This identifies
E™ = Honu/i// (PP ® Tor^i*, A), N)
with L/I acting diagonally in ?, ® Tor^A, A). By Lemma 34.1 the differential
in P. makes P. ® Tor^(A, A) into a UL/1-ivee resolution of M ® Tor^(A, A).
This gives
E™ = ExtP L/I (M ® Tor^(A, ?), ?) ,
as desired.
(c) Coefficients in UL.
In this topic we suppose that the graded Lie algebra L satisfies:
L = L>i and each Li is finite dimensional.
It follows from this that UL is a graded vector space of finite type.
Let / C L be an ideal and choose a graded subspace W C UL so that mul-
tiplication in UL defines an isomorphism W <S> UI J=L> UL of right UI modules
(Corollary to Proposition 21.2). The proof of the Corollary shows that the surjec-
tion UL —> UL/I restricts to a linear isomorphism W -^ UL/I. In particular,
this endows W with the structure of right L//-module.
470 34 The Hochschild-Serre spectral sequence
Now let M be any graded vector space of the form M = {Mi}i>Q. Since each
Wk is finite dimensional a natural isomorphism
Wk ® Hom(M, UI) A Horn (M, Wk ® ?//)
is given by u> ® <? ?—> h, with h(m) = w ® ^(m). Thus an isomorphism
Hom(M, ?/L) A JJ W'fc ® Hom(M, UI)
k
is defined by / ?—> (fk)·. where for m G ?, fk(m) is the component of f(m) in
Wk ®UI. (Note that given any m G M and any sequence /^ G W\ ®Hom(M, UI)
of degree ? we have ?-(??) G ??^ ® UIdegm+p_k. Thus ?-(??) = 0, k > degm + p
and the sum ? /fc(m) is finite.)
Now suppose M is a right L-module. The isomorphism above then restricts
to an isomorphism
? : Homt//(M, UL) A JJ Wfc ® Homy/(M, UI) .
A:
The infinite product contains the direct sum 0 Wk ® Homi//(M, C//) as a sub-
space, and this is just W ® Homf//(M, C//).
Recall from §34(a) that Homer/ (?/, I7L) is a right L/Z-module. Thus \\Wk®
k
Homer/ (M, UI) inherits a right L//-module structure via ?, while W has a right
L//-module structure through the isomorphism W J=L> UL/I.
Note that the inclusion W<g>Homer/(MrUI) —> fl wh ®Homer/(M, I7/) iden-
*.·
tifies each VFfc ® Homer/(M, C//) as a subspace of the infinite product. A simple
calculation gives
Lemma 34.5 If ? € L/I, w G Wk and f G Homer/(M, UI) then
(w®f)-x- (-l)d*sf a^xw -x®fe W<k ® Homer/(M, UI) .
Now let ? : ?» ^> k be a C/L-projective resolution of ic. Recall (§34(a)) that
the canonical L//-module structure in Ext^r/(i:, UL) is obtained by identify-
ing Extui(k,UL) = H (Homer/(F«,C/L)) and using the L//-module structure
induced from that in Homer/(-P*, UL).
As above, identify
Homer/(F.,C/L) = Y[wk ® Homer/(F., C//)
and hence identify
The Rational Dichotomy 471
Lemma 34.5 then implies that for ? G L/I, w G Wk and a G ExtqUI(k,UI) we
have
(w ® ?) · ? - (-l)desQ d^xw -x ® a G W<fc ® Ext^Jfc, C//) . C4.6)
It follows that W ® Exty/(A', UI) is an L//-submodule of the infinite product.
Proposition 34.7
(i) W ® Ext^Jb, UI) is a free UL/I-module on 1 ® Ext^Jfe, UI).
(ii) If either UL/I or Ex.X1qUI(k,UI) is finite dimensional then
W ® Ext^i*, UI) = Extg^Jfc, UL) .
proof: Denote Extfj^k, UI) by Eq.
(i) An L//-linear map ? from the free L//-module Eg ® [/I,// to M^ ®
given by
, ? e Eq, aeUL/I .
(We identify C/L// = IV.) It follows from formula C4.6) that ?(? ® ?) -
(_1)??8? degoa (g, ? G Pi/<dega ® ?:«. This implies that 0 is an isomorphism,
(ii) If either UL/I or Eq is finite dimensional then
= J| W/t ® ?« = Ext^Jfc, C/L) .
Recall again, that L is a graded Lie algebra satisfying L = {?t}f>1 and each
Li is finite dimensional. Suppose further that
• / C L is an ideal and that ? is an L-module.
Write UL = W ® C// as above. Then (C/L)8 = (C//)8 ® PF» and
Tor^ (TV, (t/L)*) = Tor^ (iV, {Ulf) ® W» . C4.8)
The surjection I7L —>· t/L// restricts to an isomorphism W -^ UL/I, which
dualizes to (UL/I)* -=> Ws and so makes W* into an L/I module. Thus the left
hand side of C4.8) is an L//-module because ? is an L-module (Lemma 34.3)
and the right hand side is an L//-module via the action in W".
However, C4.8) is usually NOT an isomorphism of L/7-modules. Even so,
it is often possible to construct an isomorphism that does preserve the L/I-
module structure, as the next proposition shows. Recall (Lemma 34.3) that
each TorJ^jV, A) is an L/J-module.
472 34 The Hochschild-Serre spectral sequence
Proposition 34.9 With the hypotheses and notation above assume the Lie
algebra L/I is finitely generated and that a · UL/I is finite dimensional for each
a G Tor^!(N, He). Then there is an isomorphism of U L /1-modules
Tor^ (TV, (ULf) Si Tor^ (N, {Ulf) ® (UL/lf
where L/I acts on the right via the action in (UL/lf. In particular,
(Jfc, TorJ" (N, (ULf)) = Tor^' (Jk, (UL/I)*) ® Tor^ (N, (Ulf) .
Lemma 34.10 With the hypotheses of Proposition 34.9, ? · UL/I is finite di-
mensional for all ? G Tor^ (N, (ULf).
proof: Write M = (ULf. Then M = M<0 is the union of the submodules
M>-p. It is thus sufficient to prove the lemma for ? e Tor^ (N, M>~p). Con-
sider the exact sequence
TorJ" (N, M>_p) -> Tor^ (N, M>_p) -> Tor^ (?, ??_?) ,
where UL acts trivially in M_p. By hypothesis the lemma holds for this trivial
C/L-module. Hence for ? e Tor^ (Ar,M>_p) and some ? > 0, ?-(UL/I)>n c
Image Tor^ (N,M>-P). Because L/I is a finitely generated Lie algebra each
(UL/I)>n is a finitely generated t/L//-module. Hence so is ? -(UL/I)>n. By
induction on ? it follows that ? -(UL/I)>n is finite dimensional. Hence so is
? · UL/I. a
proof of Proposition 34.9: Dualize the inclusion UI —> UL to a surjection
(ULf —> (Ulf of [//-modules. This induces a linear map
/ : Tor^ (TV, (ULf) -> Tor^ (N, (Ulf) ,
and C4.8) shows that / is surjective.
Right multiplication in UL/I makes Uom(UL/I, —) into a left (and thus right)
UL/I-modu\e. Define a morphism
F : TorJ" (TV, (ULf) —> Horn (UL/?,???^1 (N, (Ulf))
of UL/I-modules by setting F(a)(a) = f(a-a), a € TorJ" ((N, (ULf), a G
UL/I. Recall that an inclusion
? : TorJ" (N, (Ulf) ® (UL/lf —> Horn (UL/I, TorJ" (iV, (C//)»))
of C/L//-modules is given by ?(? ® g)(a) — {g.a)a. the image of ? consists
of the linear maps ? : UL/I —> ???^1 (?,(Ulf) such that for some ?(?), ?
The Rational Dichotomy 473
vanishes on (UL/I)>n, y Thus Lemma 34.10 implies that Im F C Im ? and we
may regard F as a ?/?,/7-linear map into Tor^ (N, {Ulf) ® {UL/lf.
Finally, identify {UL/If = Wi and use C4.8) to write F as a map
F : ???^1 (?, {Ulf) ® W" —> Tor, (TV,
This F may not be the identity, but a straightforward calculation shows that
(F - id) : — ® Wtn —> - <S> W>_n> ? > 0. Thus F is an isomorphism. ?
Exercise
Suppose / is an ideal in a graded Lie algebra L = L>\ and that each Li is finite
dimensional. Prove that:
(a) If Ext^iJb, UI) = 0, j < q, then Ext{,L(A, UL) = 0 , j < q .
(b) If in addition Ext{//(A, UI) is finite dimensional for q < j < ? + q + 1 and
if Ext\jL/I(&, UL/1) = 0 for i < ? then
k JO ,k < p+ q
ExtUL(lk, UL) = \ /n-o>T?„*9 (IL- TTT\ b-n-t-n
\ih, ? ? ) ,?. — ? ? q ·
35 Grade and depth for fibres and loop spaces
In this section (unless otherwise specified) the ground ring k is an arbitrary
principal ideal domain.
Recall from §20 the functors Ext^(-,-) defined for right modules over a
graded algebra A, and the definition of projective dimension of an yl-module.
Definition 1. The grade, grade^(M), of a right rl-module is the least integer
k such that ExtkA(M, ?) ? 0. (If Ext*A(M,A) = 0 we say grade^(M) = oo.)
The projective grade, projgradej4(M), is the least integer k (or oo) such that
ExtkA(M,?) ? 0 for some yl-projective module P.
2. If A = {Ai}i>0 and Ao = k then the depth of .4, depth A, is
the grade of the trivial yl-module k. The global dimension of A, gldimyl is the
greatest integer k (or oo) such that ExtA(k, -) ? 0.
The main theorem of this section provides a connection between LS category
and the homological notion of grade. Recall from §2(c) that if
f:X—>Y
is a continuous map then a holonomy action of the loop space ? F is determined
in the homotopy fibre F of /. This makes Ht(F) into a right Ht(UY)-modu\e.
We shall prove:
• If X is normal and (Y,yo) is well based, and if Ht(F) and ??(??) are
k-free then
projgrade^(fJy) Ht(F) < cat/ .
Moreover, if equality holds then
H*(F) = cat/ = projdim^ny) H*(F) .
When each Hi(F) and Hj(QF) have finite k bases we will replace projgrade by
grade in this theorem.
The special case that / = id : X —> X is sufficiently important that we
restate the theorem for it:
• If X is path connected, well based and normal and if Ht(QX) is k-free
with a finite basis in each degree then
depthH*(nX) <catX .
// equality holds then depth ?.(??) = catX = gldimH.(ClX).
The depth theorem for topological spaces was originally deduced, for the case
that k = Q and Ht(X) has finite type, from a theorem on Sullivan algebras
established in a joint paper with Jacobsson and Lofwall [54]. Subsequently it
was extended for spaces to Jc = Fp (with H»(X) still of finite type) in a joint
The Rational Dichotomy 475
paper with Lemaire [85] using a different but similar approach. However the
theorem for Sullivan models in [54] is for a coefficient field of any characteristic,
and is applied as such to the Ext-algebra of a local commutative ring.
The proof given here (for the more general grade theorem) is different in form,
although based on the same underlying idea. We shall, however, also sketch the
proof of the Sullivan algebra theorem in characteristic zero, since it provides an
interesting application of the material in §29:
• If L is the homotopy Lie algebra of a minimal Sullivan algebra (AV, d) and
if V = {V*} is a graded vector space of finite type then
depth UL < cat(AV, d) < gl dim UL .
Moreover, if depth UL = cat(AV, d) then cat(A V, d) = gl dim UL.
Note: When (AV, d) is the Sullivan model of a simply connected space X, then
UL = ?*(??) and cat0X = ca,t(AV,d), (Theorem 21.5, Proposition 29.4) and
so the two results coincide.
This section is organized into the following topics:
(a) Complexes of finite length.
(b) fiy-spaces and C,(fiF)-modules.
(c) The Milnor resolution of Jk.
(d) The grade theorem for a homotopy fibre.
(e) The depth of H.(flX).
(f) The depth of UL.
(g) The depth theorem for Sullivan algebras.
Both the grade theorem and the Sullivan algebra theorem have their roots in
an elementary theorem about ? (Hom^(F,, Q»)), where A is a graded algebra,
P, is an yl-projective resolution of an ^4-module M and Q» is a complex of free
yl-modules of finite length. Topics (b)-(e) are then devoted to the grade theorem,
which is proved in a topological setting with no reference to Sullivan algebras or
graded Lie algebras. This material can be read with only Part I, Part III and
§27 as background. Topics (f) and (g) deal with the Sullivan algebra result.
(a) Complexes of finite length.
Let A be a graded algebra (over A). As in §34 we denote by F, = {F;} a chain
complex of yl-modules Pi = Pi,* of the form
0 *-Po,. ?¦ Pi,. *
476 35 Grade and depth for fibres and loop spaces
in which (Fj,.) ¦ = Pij-i- If Q* is asecond such chain complex then Hoiru(P., Q*
will denote the bigraded complex of ic-modules given by
????(?.,?.){,. =\{RomA{Pj,Qj+i) ,
i
with df = dof- (-l)dezff ? d.
Key to the proof of the grade theorem is
Lemma 35.1 Suppose P. -—> M is an A~projective resolution of an A-module
M and suppose Q* = {Qi}o<i<m iS a complex of free A-modules. Then
Hi^ (Hom^(P,, Q.)) = 0 , i> m- proj grade^ M .
proof: Set Q't = {Qi}o<i<m-i· Because the Pi are /1-projective the sequence
0—>Hom.4(P.,Qt) —>Homy4(P.,g.) —> HomA(F., Qm) —> 0
is exact. Since Qm is ?-free, i/;,. (Hom^(P., Qm)) = Ext™'*(M,Qm) = 0,
i > m — projgrade^ M. By induction on m, //j,, (Hom^iP,, Q',)) = 0, i >
m - proj grades M. The lemma follows. D
Suppose next that an ?-free module ? has an A-basis xa with only finitely
many elements in each (ordinary) degree and that A and ? are concentrated in
degrees > 0. It is then immediate that
EomA(-,N) = Y[xa.EomA(-,A) ,
a
whence
Thus we have
Lemma 35.2 Suppose in the situation of Lemma 35.1 that A and Qi are con-
centrated in nonnegative degrees, and that each Qi has an ?-basis with finitely
many elements in each degree. Then
Hi,. (Honu(P., Q*)) = 0 , i>m- grade^ M .
(b) fir-spaces and C.(fir)-modules.
Let (Y, y0) be a based path connected topological space. Multiplication in
the loop space ?.? makes it into a topological monoid (§2(b)). A topological
space ?' equipped with a right ??-action will be called an QY-space and a map
The Rational Dichotomy 477
of ??-spaces is a continuous map that preserves the action. If X and ? are
QF-spaces then UY acts diagonally on ? ? ? via (x, z) · 7 = (x · 7, ? · 7).
Next recall (§8(a)) that multiplication in ?? makes ?*(??) into a chain
algebra via the Eilenberg-Zilber equivalence,
c.(nr) ® c.(nr) ^> c.(nr ? ??) c'(mult)> c.(ny).
In the same way, if Ar is any fiF-space then the action defines a C,(fii')-module
structure in C»(X). For example, the constant map ?? —y pt defines a chain
algebra morphism C*(QY) —> k, and makes k into a C,(fiF)-module, because
C,(pt) = k — cf. D.2).
Now consider the Alexander-Whitney comultiplication
? : c.(nr) —» c.(nr) ® c.(nr)
introduced in §4(b). It follows directly from the compatibility D.9) of the
Eilenberg-Zilber and Alexander-Whitney equivalences that ? is a morphism of
chain algebras, and so it makes ?*(??) into a differential graded Hopf algebra
(as also described in §26(b)). In particular, if (M,d) and (N,d) are any two
C„(fir)-modules then (M,d) ® (N,d) is a C»(QY) ® Ct(UY)-modu\e in the
obvious way, and this action composed with ? makes (M, d) ® (N, d) into a
C.(CIY)-module. We call this the diagonal action of C*(QY).
Now suppose X and ? are fiF-spaces, and let ?,? act diagonally on ? ? ?.
A simple calculation with formula D.9) gives
Lemma 35.3 The Alexander-Whitney equivalence
AW :C.(IxZL C»(X) ® C.(Z)
is ? quasi-morphism of ?»{??)-modules, with respect to the topological and al-
gebraic diagonal actions. ?
Next, we pass to homology. For any complexes (M,d) and (N,d) a natural
map
H(M, d) ® H(N, d) —>H ((M, d) ® (iV, d))
is defined by sending [z] <S> [w] 1—y [? <S> w] for any cycles ? and w in M and iV.
Moreover, if M and H(M) are ic-free (or if iV and H(N) are A-free) then this is
an isomorphism. Indeed, because d(M) C M and k is a principal ideal domain,
Im d is jfc-free. Split the short exact sequences
0 —» ker d —» M —» d(Af) —> 0 and 0 —» Im d —» ker d —> ff(M) —> 0
to write (M,d) s (f/'(M),O)e0(Amu,JK;dmu). Since H(M)'\s ic-free we obtain
H((M, d) ® (iV, d)) = H{H{M)® (N, d)) = ff(M) ® ff(JV).
In particular the map
if(mult)>
478 35 Grade and depth for fibres and loop spaces
makes ?*(??) into a graded algebra and, if X is an ??-space, then the map
> ? {Ct{
makes Ht(X) into a right f/,(fiF)-module.
Now suppose #.(??) is Jc-free. Then we may use ?*(??) ® ?*(??) =
?(?*(??) ® ?7.(??)) to identify #.(?) as a morphism
ff.(A) : ?.(??) —>
of graded algebras; in other words, ?*(??) is a graded Hopf algebra. Thus
if X and ? are ??-spaces then H*(CIY) acts diagonally on H*(X) ® H*{Z)
and, moreover, the morphism H*{X) ®HM(Z) —> ? (C*(X) ® C*(Z)) is a mor-
phism of f/,(fiF)-modules. In particular if Ht(X) is ic-free then this provides
isomorphisms
Ht{X)®H*{Z) ^ H{Ct{X)®C»{Z)) <—-—i/»(X ? ?)
H(AW)
of ff.(nr)-modules.
(c) The Milnor resolution of .fc.
Let (F, yo) be a well based path connected topological space. For topological
groups G, Milnor [125] constructed the universal bundle EG —> BG by putting
EG = G*°°, the infinite join of G with itself (§2(e)). Here we consider the
topological monoid ?,? and, when ?*(??) is It free, we use the filtration of
(??)*°° by the subspaces (??)*? to construct an Eilenberg-Moore resolution of
the C*(fiF)~module, Ik (§20(d)), which we call the Milnor resolution.
Recall from §l(f) that the join X * ? of topological spaces is the space (? ?
CZ) U(CX x Z). If X and ? are fiy spaces then ?? acts diagonally on ?*? in
the obvious way and the inclusion X —> ? ? {0} in X * ? is a map of ?? spaces.
In particular, starting with the action of ?? on itself by right multiplication we
obtain a diagonal action in each of the joins (??)*? = (??)<?~^ * ??. The
inclusions
?? —> (??)*2 —> »· (??)*? —>
are maps of fiy-spaces and, as in §2(e), we set (??)*°° = 1](??)*? with the
?
weak topology determined by the (??)*?.
Lemma 35.4 Let A C X be an inclusion of ??-spaces and give (?, ?) ? ??
the diagonal action, where ?? acts by right multiplication on ??. If H*(X,A)
is It-free then ?. ((?,?) ? ??) is Hm(QY)-free.
proof: If 7 6 ?? is a loop of length ? let 7' be the loop of length ? given by
7'(f) = ?(? — t), 0 < t < ?. Then 7 1—> 77' and 7 1—> ?'? are homotopically
constant maps. Thus the map
f-.????^????, /(x,7) = (x-7,7)
The Rational Dichotomy 479
is a homotopy equivalence with homotopy inverse (x, 7) 1—> (? «7', 7).
Denote by (X ® ??)? and (? ? ??)? the ??-spaces in which ?? acts
respectively diagonally and by right multiplication on ??. The isomorphism
(cf. §35(b)) H.(X,A) <8> ?.(??) s H. ((X, A) ? ??)? identifies this latter as
the free H*(QY)-module with basis a J;-basis of H*(X,A). On the other hand,
/ : (? ? QY)r —> (? ? ??)& is a map of ??-spaces and Ht(f) is therefore
an isomorphism of i/«(fiF)-modules. G
Lemma 35.5
(i) (??)*°° has the weak homotopy type of a point,
(ii) ?/?.(??) is k-free so is each ?* ((??)*?).
proof: (i) This is an easy exercise since ?? is well-based (Step 1 of Propo-
sition 27.9) and so (??)*<?+1) ~ ?(??*? ? ??) of §l(f), and (V
(ii) When X and ? have ic-free homology the isomorphism Hm(X) <8>
H.(Z) A H{C*{X) ®-C.(Z)) -=> H.(X x Z) identifies H.(X ? ?) as Jfc-free.
Now use the formula (?>')*? ~ ?^^
Henceforth we assume ?*(??) is k-free. We are ready to construct the
Milnor resolution. Define JHxee graded modules V(n), ? > 0 by setting
V@) = Vb(O) = k and V{n) = H* (C(QY)*n , (??)*?) , ? > 1 .
Set V = 0V(n). The Milnor resolution will have the form (V <g> C*(QY),d)
?
with d : V(n) —> V(< n) ® C*(UY) and C*(QY) acting by multiplication from
the right. We
construct it by simultaneously constructing the differential d and a commutative
diagram
··· V(<n)®C*(QY) ···
in which the vertical arrows are quasi-isomorphisms of C«(fiF)-modules. '
Indeed, set ?@) = id and then suppose by induction that ?(? — 1) and the
differential in V(< n) ®C*(UY) are constructed- Use excision and Lemma 35.3
to identify
480 35 Grade and depth for fibres and loop spaces
?, ((??')·(?+1),(????) = H {{C{C\YYn,{UYYn) ? UY) as the free H.CiY)-
module V(n)®H*(UY).
Next, let va be a basis of V(n) and choose elements za e C, ((??)*(?+1))
such that
• za maps to a cycle in C* ((??)*(?+1),(??)*?) representing vQ,
and
• dza = ?(? — l)uQ for some cycle ua e V(< n) ® ?7«
Extend the differential to V(< n) ® C,(?F) by setting dvQ = uQ and extend
?(? — 1) to ?(?) by setting ?(?)?? = zQ. Then <^(n) induces a quotient chain
map ?(?) : V(n)®Ct(UY) —> C, ((??)^^1),(??)*?), and i/((^(n)) is the
identity, by construction. By the five lemma 3.1, ?(?) is a quasi-isomorphism.
Thus the morphisms ?{?) define a quasi-isomorphism ? : (V ® C«(?l/'),d) -^>
C, ((??)0), while the constant map (??)*00 —)¦ pi is a map of ??-??????
and a weak homotopy equivalence (Lemma 35.5). Composing yields a quasi-
isomorphism of C ^F)-modules
(F®C,(fir),(iLl, C5.6)
which we call the Milnor resolution of k.
Filter this semifree resolution by the submodules (V(< n) ® Cm(UY),d) to
obtain a spectral sequence whose ??-term has the form
0 ^-Jc ^-H*(UY) < ^-V(n)®H*(UY) ^- . C5.7)
Proposition 35.8 The sequence C5.7) is an Ht(QY)-free resolution of Jk;
i.e., the Milnor resolution is an Eilenberg-Moore resolution (%20(d)).
proof: We need only show C5.7) is exact. Filter C, ((??)*°°) by the submod-
ules C, ((??)*?). Then the quasi-isomorphism ? : V®C*(QY) —> C, ((?>')*°°)
preserves filtrations. In the construction of ? we showed that each ?(?(?)) was
an isomorphism. This means precisely that ? induces an isomorphism between
the i?1-terms of the spectral sequences. It is thus sufficient to prove that
k <— Hr(QY) A ¦¦¦?-!
is exact.
Suppose by induction that
0 <_ jfe <_ ?.(??) A · · · ?- ?*
is exact. A simple spectral sequence argument then shows that any d\ -cycle, a,
in
The Rational Dichotomy 481
?. ((??)*?,(??)*(?-1)) is the image of some class ? € Ht ((??)*?). But
(?.?)*? is contractible in (??)*(?+1). Thus a representing cycle, z, for ? has
the form ? = dw for some w € C« ((??)*(?+1)). In particular, u> represents a
class 7 € #, ((??)*(?+1\ (??)*?) and di7 = <*· This closes the induction and
completes the proof. D
(d) The grade theorem for a homotopy fibre.
Fix a continuous map
/ : X -> Y
from a normal topological space X to a path connected, well based topological
space (Y,y0). Convert / into the fibration ? : ? ?? MY —> Y, whose fibre
F = X xY PY is the homotopy fibre of / (§2(c)). The action of ?.? on F then
makes H.(F) into an Hm(QY)-mod\ue (§35(bj).
Theorem 35.9 (Grade theorem) With the hypotheses and notation above sup-
pose H»(F) andHm(UY) are k-free. Then
(i) projgradeH.(nK) H*(F) < cat/.
(ii) If equality holds in (i) then
*(F) = cat/ =
proof: Since proj grade < proj dim by definition, both assertions are vacuous
unless cat / is finite. Set cat / = m. The proof of the theorem is in five steps.
Step 1: We construct a map
of UY-spaces, where UY acts diagonally on (QY)*^m+^ as described in §35(b)).
For this recall the construction in §27(c) of the Ganea fibrations pm : PmY —>
Y, with fibre Fm at y0. According to Proposition 27.8 (with the roles of X and
Y reversed) there is a continuous map ?0 : X —> PmY such that pmao = f ¦
Moreover, by construction PmY is obtained by convertingPm-iYuCFm-i —> Y
into a fibration and so there is a homotopy at from ?? to a map ?\ : X —>
Pm-\Y U CFm_i. Define a continuous map
9'.XxYMY—>PmY = (Pm-iY U CFm_x ) ? ? MY
as follows: if (x,w) € ? ?? MY and w is a path of length ? then 9(x,w) =
(???,??1), where w' is the path of length i + 1 given by w'(t) = pmai_t(x),
0 < t < 1 and w'(t) = w(t — 1), t > 1. Observe that pm9 = p, and so ? restricts
to a continuous map
to a continuous map
482 35 Grade and depth for fibres and loop spaces
By construction, 9f is an QY-map.
On the other hand, Proposition 27.6(ii) provides a map Fm —> Fm-.x * ?.?
which is a map of QF-spaces and a homotopy equivalence. Iteration of this con-
struction produces an ?,?-map Fm —> (fi7)"(m+1>, because Fo = QY. Compo-
sition with ? F produces the desired hF : F ^l
Step 2: We construct an algebraic model for the continuous map
h = (id, hF):F^F ^)
Choose an Eilenberg-Moore resolution (Proposition 20.11), a : (R,d) —t
C.(F), for the C,(fir)-module C*(F) described in §35(b). Then recall the Mil-
nor resolution ? : (V ? C*(UY),d) A C. ((??)*°°) of §35(c) and denote the
obvious inclusions by Am : (V(< m) ® C*(UY),d) —+ (V ® C.(UY),d). The
algebraic model of h we wish to construct will be a morphism
of C«(fiF)-modules, where C*(UY) acts diagonally on the target, and it will
satisfy:
(Am <g> ??)? : (R,d) —> C,(F) ® (V ® C,(fiF),d) zs ? quasi-isomorphism.
In fact, since (A, d) is semifree we may lift morphisms from (R, d) (up to
homotopy) through quasi-isomorphisms (Proposition 6.4(ii)). Apply this remark
to the diagram
to construct ?. (Note that AW is a morphism of C«(fiy)-modules by Lemma 35.3.
To show (id®\m)%lj is a quasi-isomorphism, let jm : (CiY)*(m+1'> —> (??)*00 be
the inclusion. Then
(id x jmOi = (id,jmhF) :F^Fx (??)*°°
is a weak homotopy equivalence, because (??)*°° —> pt is (Lemma 35.5(i)). But
(idxXm)ip is connected (up to homotopy) to the quasi-isomorphism C« ((id ? jm)h)
by quasi-isomorphisms, and hence is one itself.
The Rational Dichotomy 483
Step 3: The associated H*(IIY)-free resolutions of Ht(F).
In the Eilenberg-Moore resolution (R,d) -=> C*(F) write R = W <8>C*(QY)
oo
where: W is the direct sum W = 0 W(p) of ic-free modules, d : W(p) —>
p=0
W(< p)®C.(nr) and the filtration {W(< ?) ® ?.(??)} is the Eilenberg-Moore
filtration of R. This defines a homology spectral sequence (Ei,di) whose E1-
term by definition gives an ?(??)~(??? resolution of H*(F) of the form
H.(F) <— W@) ® ?.(??) ?- W(l) ? ?*
We denote this resolution by
P. -?> Jf.(F)
with (as in §35(a)) Pp =
Next, filter C(F) ® (V (g)C,(fir),d) by the submodules C,(F) ® T^(< ?) ®
C,(??). This produces a second homology spectral sequence whose E1-term
gives a complex of u«(fiF)-modules of the form,
Ht{F) <— Hm(F)®V@)®H.(nY) ?- H.(F) ®VA) ® H.(QY) ? ,
since H.(F) and ?.(??) are it-free (cf. §35(b)). Now ?.(??) acts diagonally
in (H.(F) <S> V(p))®H*(QY) and this is a free #»(i7y)-module on a it-basis of
H*(F) ® V(p) (§35(b) and Lemma 35.4). Moreover, since the Milnor resolution
is an Eilenberg-Moore resolution (Proposition 35.8) it follows that this sequence
is exact; i.e. it is also an H*(QY)-iree resolution of H*(F). We denote it by
with Qp = H.(F) ® V(p) ® ?.(??).
Step 4-' Set projgrade^^yj H*(F) — r. Then ? is homotopic (as a morphism
of C*(QY)-modules) to a morphism ? such that
?: W(p) ^> Ct(F) ®V(< ? + m - r) ® C*(UY), p>0 .
Filter C„(F)(g>V(< m)®C*(UY) by the submodules C. (f)® V{< p) ®C. (??)
to produce a spectral sequence whose E1-term is just (Q<m,di), and denote all
spectral sequences by (E\dl).
k
Now write ? as the infinite sum ? = J2 ?? of the C»(fiy)-linear maps ??
—oo
defined by
484 35 Grade and depth for fibres and loop spaces
(Necessarily k < m.) Then ????? = i>kdo and H(ipk) is an Ht(QY)-lineax map
that raises the resolution degree by exactly k. We may now apply Lemma 35.1. It
asserts that if k > m-projgradeH-(Qr) H*(F) (= m-r) then H(ipk) = ???+??}
for some H*(ClY)-linear map ? : ?« —> Qt that raises resolution degree by k + 1.
Choose C„(ttr)-linear maps ?' : W(p) ® C*(QY) —> C,(F) ® V(p + k +
1) ® C*(QY), ? > 0, so that 6>'(it; ® 1) is a cycle representing 9(w ® 1). Then
do9' + 9'd0 = 0 and hence ?' = ? - (??' + 9'd) also raises filtration degree by at
most k : ?' = ??'??·
i<k
By construction, H(tp'k) = 0 : P« —> Q<m- Choose C«(fiF)-linear maps
?" :W(p)®C.(ttY) ^ C4F)®V(p + k)®C*(UY), ? > 0, so that do9"(w®l) =
ip'k(w®l), w €W. Set ?" = ?'-(??" + ?"?). By construction, ???" + ?"?? = ^
fc-l
and so ?" = ? ?", while clearly -?" ~ -?.
Remark If the ic-modules Hi(F) and /?^(??) have finite bases for each i and
j then this will also be true of each [H*(F) ®V(< m)]k, as follows from the
definition of V in §35(c). In this case we may use Lemma 35.2 in the proof of
Step 4 to find ? ~ ? with
? : W(p) —»· C,(F) ® V(p + m - ?) ® ?(??) , ? > 0 ,
where ? = gradeH_(QK) H.(F).
Step 5: Completion of the proof of Theorem 35.9.
Suppose projgrade^^Qyj H.(F) > cat/ and, as above, write m = cat/ and
r = pro] gradefj^QY^ H.(F). Since r >m Step 4 provides a filtration preserving
morphism of C.(Oy)-modules,
? : (W ® C*(UY),d) ^ C.(F) ® (V(< m)
Thus ? induces a morphism ??(?) of spectral sequences which, at the i?1-level
has the form
(in the notation of Step 3).
Compose ? with the inclusion id®Xm of Step 2 to obtain a filtration preserving
morphism
?' : (W ® C.(nr),d) —»· (C.(F) <8> (F ® Ct(QY),d)) ,
which then yields a morphism ?'(?') of spectral sequences. The E1 -terms of
these spectral sequences are respectively the resolutions (?^,?1) and (Q«,^1) of
Step 3; in particular it follows from Step 3 that in both cases E2 = -Eg». In
The Rational Dichotomy 485
each case ??, = H,(F) and so the inclusions W@) <8>G,(??) —> W®C,{QY)
and C,{F) e V@) <8> ?7,(??) —»· C.(F) ® F ® G.(?1') induce isomorphisms
?"o,* —¦> H(—) which identify ?2(?') — ?^,(?') = ? (?1).
But 77' = (id ®AmO7 ~ (id® Am)t/>, which is a quasi-isomorphism by Step 2.
Hence ?2(?') is an isomorphism and
is a quasi-isomorphism of resolutions.
Suppose first that projgrade^^y) H*(F) > cat/. Then Step 4 would allow
us to choose ? so that ? = ? Vk- It would follow that ??{?) = 0 and so
fc<0
Ex{q') — E1(id<8>\m) ? ?1 (?) = 0 as well. Since ??(?') is a quasi-isomorphism
this is absurd; i.e.,
H.(F) < cat/ .
Now suppose projgradeH(QK) H*(F) = cat/, and let JV be any right H,(QY)-
module. Since E1^') is a quasi-isomorphism of resolutions, it induces a quasi-
isomorphism
Hominy)(P., ??) <=- Homw.(ny)(C,,iV) .
It follows that ?1 (?) induces surjections
H"'" (EomHAnY)(Pt,N)) «- H™ {UomHm(nY)(Q<m,N)) ,
where (as in §35(a)) the left degree "p" is the resolution degree.
Since Q<m is concentrated in resolution degree < m and since ? is concen-
trated in resolution degree zero it follows that Hp'* (Hominy)(?,,./?)) = 0,
p> m. But
HP'* (EomHAnY)(Pt>N)) = Extp {H.{F),N), since P. is an H.{QY)-free reso-
lution of H* (F) (cf. §20(b)). Thus ???^"(??) (Ht(F),-) = 0. Since, by assump-
tion, projgradeH>(Qr)u,(F) = m it follows that ???^(??) (H*(F),-) ? 0.
Thus projdim/i^ny) (Hm(F)) = m, as desired. This completes the proof. ?
Corollary: Assume, in addition to the hypotheses of Theorem 35.9, that the
Jz-modules H{(F) and Hj(QY) have finite bases for each i and j. Then
gradeH.(QK) H.(F) < cat /
and if equality holds then also cat/ = projdimjy^ny) H*{F).
proof: The Remark at the end of Step 4 in the proof of the theorem allows us
to replace proj grade by grade in Step 5. ?
486 35 Grade and depth for fibres and loop spaces
(e) The depth of ?.(??).
Theorem 35.10 If (X,x0) is a normal path connected topological space and if
each Hi(QX) is Ik-free on a finite basis then
depth?.(??) < catX .
// equality holds then also cat.Y = g\dimHt(QX).
proof: Replace X by a well based space of the same homotopy type by adjoin-
ing an interval to X at the base point x0. Then apply the Corollary above to
Theorem 35.9 to / = idx. D
Corollary If h is a field of characteristic zero and if X is simply connected
then depth ULx < cato X, where Lx is the homotopy Lie algebra.
proof: Replace X by the rationalization (§9) Xq and note (Theorem 21.5 and
Proposition 28.1) that ULX = H*(ilX;Jc) = ?*(??®;1?) and that cat0 X =
?
(f) The depth of UL.
In this topic Jk is an arbitrary field of characteristic zero.
Suppose L — {Li)i>\ is a graded Lie algebra, with universal enveloping al-
gebra UL. In §22(a) and §22(b) we constructed C*(L;UL) and established its
properties, where L is regarded as a differential graded Lie algebra with zero
differential. This gives, in particular, a UL-iree resolution of Ik of the form
k*—UL<±-sL®UL4 4- AnsL ®UL <
This resolution, which plays the key role in this and the next topic, will be
denoted by
P. ^> Ik .
By definition (§20(b)), Ext^L(A, -) = if"·· (Hom^F,, -)).
Proposition 35.11 Let L = {?;};>1 be a graded Lie algebra with each Li finite
dimensional.
(i) gldim UL is the largest integer ? (as ooj such that Exi^L(Jc, k) ? 0.
(ii) depth UL < gl dim UL.
proof: (i) Clearly ? < gl dim UL, since ExtyL(A, Ik) ? 0. On the other hand,
write F, = C*(L) ? UL. Then
ExtUL(Jc,k) = H(HomUL(P*,]k)) = ? {C*(LN) = ? {C*{L)Y ,
The Rational Dichotomy 487
because k is a field. It follows that H(C*(L)) is concentrated in homological
degrees < n.
Now choose a graded subspace ? C AnsL so that E®d (An+1sL) = AnsL.
Observe that (A<nsL 0 E) <8> UL is automatically a subcomplex of P«, and that
the inclusion defines a morphism of the spectral sequences derived from the fil-
trations Fp(—) = (—) •(UL)>p. At the ?7°-term the differentials are just d <8> id
in C*(L) <g>UL and so this morphism is a quasi-isomorphism of ?7°-terms. It fol-
lows that the inclusion of (A<nsL © E) ®UL in P» is itself a quasi-isomorphism.
This (A<nsL © E) <g> UL is a t/L-free resolution of 1; and so Ext?2(&> -) = 0;
i.e., gldim UL < n.
(ii) Let gldim UL = n. Then Ext^1 (¦&> ~) = 0. Apply B.omUL(P*, -)
to the short exact sequence 0 —> (UL)+ —> UL —> 3: —> 0 and pass to
homology to obtain an exact sequence
Ext&i (A, t/L) -»¦ Ext&L(A, A) -»¦ Ext^1 (*, (t/^)+) ·
Thus ???^?,(?, I7L) surjects onto Ext^?,(A, A), which is non-zero by (i), and so
depth UL < n. ?
(g) The depth theorem for Sullivan algebras.
As in the previous topic, in this topic Ik is an arbitrary field of characteristic
zero.
Fix a minimal Sullivan algebra (AV,d) such that V = {^*}i>2 is a graded
vector space of finite type, and let L be the homotopy Lie algebra of (AV, d) as
defined in §21 (e). Recall that cat(AV, d) is defined in the introduction to §29. As
in the previous topic the complex P» = C*(L; UL) and associated constructions
will play a key role.
In particular, let d\ denote the quadratic part of the differential in AV : d\ :
V —> A2V and d-di : V —> A^3V. From the definition of the Lie bracket in
§21(e). and Proposition 23.2, and Example 1 in §23(a) we obtain
) = C*(L) = EomUL(P*,]k) ,
with A?V = HomUL(Pp,]k). Thus
ExtpUL(k,]k) = Hp'm(hV,d{) ,
with the left degree "p" corresponding to wordlength in AV.
Proposition 35.12 cat(AV, d) < gl dim UL.
proof: Let ? = gldim?/L; according to Proposition 35.11 it is the largest
integer such that Ext?L(A,A) ? 0. Define an ideal / C AV by setting / =
A>nV ? In. where ? @ (kerdx)"·* = AnV. Then H(I,di) = 0. Filter / by
wordlength and use the associated spectral sequence to deduce that H(I,d) — 0.
Conclude from Corollary 2 to Proposition 29.2 that cat(AV, d) < nil(AV//) < ?
because (Av, d) —>. (Av//, d) is a quasi-isomorphism. D
488 35 Grade and depth for fibres and loop spaces
Theorem 35.13 [54] // L is the homotopy Lie algebra of a minimal Sullivan
algebra (AV, d), and ifV= {V'}i>o is a graded vector space of finite type, then
depth UL < cat(AV, d) < gl dim UL .
Moreover if depth UL = cat(A V, d) then cat(AV, d) = gl dim UL.
proof: In view of Propositions 35.11 and 35.12 it is sufficient to prove that
depth UL > cat(AV,d) =*> cat(AV,d) > gldimt/L ,
and for this we may suppose cat(AV, d) is a finite integer m.
Recall first that in §29(f) the surjection (AV,d) —> (AV/A>mV,d) is extended
to a (AV", d)-semifree resolution of the form
? : (AV ® (Af © A), d) -^> (AV/A>m, d) ,
and with the following three properties:
• The differential di in AV extends to a differential ?\ in AF ® (? ? Je)
characterized by
- ?? : M —> (V (g) ?) ? Am+1 V, and d - ^ : M —> (A^2V ® M) ©
>+2
- AV (g) (M ? Je) is a (AV, d^-module.
• The surjection (AV,di) —> (AV/A>mV,d1) extends to a (AV,dx)-semifree
resolution
? : (AV ? (? ? Jfe),Ai) ^> (A\7A>mV, di)
such that ?(?) = 0.
• Because cat(AV, d) = m there is a morphism (retraction)
? : (AV ® (M © A), d) —»· (AV, d)
of (AV, d)-modules such that ?A) = 1.
Now bigrade AV and the tensor product by setting (AV)P>* = APV and
(AV ® (M © Jk))p'* = (Ap-mV®M) ? ApV. Then filter by setting Fp(-) =
(—)-Pi*. Thus d\ (or ?\) increases filtration degree by 1 while d — di (or d — ?\)
increases it by at least 2. On the other hand, ? need not preserve the filtration
degree. However ? can decrease the filtration degree by at most m and so it can
oo
be written as the infinite sum ? = ? ??? some k < m, of the AV-linear maps
i=-k
?? characterized by:
• ?? increases filtration degree by exactly i.
The Rational Dichotomy ,o„
It is immediate that ???-k ~ ?-kai = 0. Simplify notation by writing
? = ? ? ic. Then ^_fc is a cycle of bidegree (-k,0) in the bigraded com-
plex ?.???\?(?? ® ?, AV), (defined with respect to the differentials di and ??).
We show below that
Hp>*(EomAV(AV®N,AV)) =0 , p<0. C5.14)
This implies that if k > 0 then ?-k = ??? + ??? for some AF-linear map
?: AV ® iV —> AV that decreases filtration degree by exactly k + 1. Thus
^ — (do + od) is a retraction which decreases filtration degree by at most k — 1.
Proceeding in this way we find a retraction ? that preserves filtrations. But
then ? = ? ?{ and ?0 : (AV ® ?,??) —> (AV.di) satisfies 770A) = 1. This
{=0
implies that ?(?0) is surjective. Since
HP>*(AV ® ?,??) -=-» #p'* (Ay/A>mV')d1) = 0 , ? > m ,
it follows that Ext??(Jfe,Jfe) = i/>m'*(AF)d1) = 0; i.e., cat(AV,d) = m >
gl dim ?/L.
? remains to prove C5.14)- We shall work entirely in the category of bigraded
complexes; in particular, Horn will always mean bigraded Horn and j{ will mean
the bigraded dual: (<?!,„)?'* = C^q.
Now regard each ApsL ® UL as a left L-module by setting
x-a= -(-l)desidegaa.x , x€ L, a€ ApsL®UL .
Denote the differential in each C*(L;ApsL® UL) by di and denote the maps
C. (L;ApsL ® t/L) -^4 C, (L; Ap~lsL ®
by 92. Then d = di +d2 is a differential of bidegree (-1,0) in the bigraded module
C„(Z,)<g>C„(L)(g>t/L, where the bigrading is given by (-)p>, = 0 Ci®Cj®UL.
As in §22(b) this complex-is in fact a differential graded coalgebra. Moreover,
if ? is the comultiplication in C*(L) then an easy calculation shows that the
composite C*(L) A C*(L) <g> C*(L) c—^ C,(L) <g> C,(L) ® C/L commutes with
the differentials and is in fact a dgc quasi-isomorphism.
Dualizing we find that multiplication in (AV, dx ) extends to a cochain algebra
quasi-isomorphism of the form
(AV®AV®(UL)Kdi) ^> (AV,di) ,
where (AV)P-* = APV and {AV ® AV ® (t/L)tt)Pl* = ? AlV ® tiV ® (C/L)8.
This is a quasi-isomorphism of (AV, di )-semifree modules and so we may apply
— to obtain a quasi-isomorphism
(AV®AVlA>mV®(UL)\di) A (AV/A>mV,di) .
490 35 Grade and depth for fibres and loop spaces
Lift this to a quasi-isomorphism into (AV <g> ?,??) and thereby reduce C5.14)
to the assertion
HP'' (HoniAV (AK ® AV/A>mV ® (?/L)«, AV)) = 0 , ? < 0 . C5.15)
Next observe that AV/A>mV ® (I7L)» = (A^msL® ?7L)*. Thus there are
canonical identifications of bigraded vector spaces
Honuv {AV ® AV/i\>mV ® (Z7L)', ??)
= Horn (AV7A>mF ® (?/L)s, AV)
= Horn ((A^msL ®UL)\ (AsL)")
= Horn (AsL, (A^msL ® E/L)M)
= Horn (AsL, A$msL ® i/L)
= Hornet (AsL ® ?/L, A-msL ® UL) ,
where we may identity (A-msL ® I7L) with A-msL ® I7L because this graded
vector space has finite type. An arduous but straightforward calculation shows
that this defines an isomorphism of complexes
HomAv (AV ® AV/A>mV ® {UL)*',AV) = KomUL(P*,P<m) .
Since AsL is a graded vector space of finite type Lemma 35.2 (applied with
A = UL and M = Jfc) gives
Hp'* (EomUL(Pt, P<m)) = 0 , ? < depth UL-m .
Since depth ?/L is supposed > m this proves C5.15) and the theorem. ?
Exercises
1. Let X be a simply connected CW complex of finite type. Prove that
depth ?. (?E3 V X); Q) = 1.
2. Let X be a simply connected finite CW complex. Prove that depth H*(QX; Ik)
for any field Ik.
3. Let X be a simply connected formal space with H*(X\Q) ~ Q[a, ?>]/
(?4,?4,?2?2) and deg ? = 2, deg ? = 2. Prove that cat0^ = 4. Consider
the map /:54VS4-+ ^(Q2,4) (resp. g : ^(Q2,2) -* ^(dj2,4)) corresponding
to the fundamental classes of the spheres (resp. to the squares of generators of
//¦•(/^(Q2,2))). Convert g into a fibration and show that X has the rational
homotopy type of the pullback of g along /.
Xo ?
E4v54)o 4
The Rational Dichotomy 491
Compute the rational homotopy Lie algebra of X using the homotopy fibre of
h. Prove that the dimension of the centre of Lx is 2, and that the depth of
Ht(QX;Q) is 3.
36 Lie algebras of finite depth
In this section the ground ring it is a field of characteristic ? 2,3.
Throughout the section we adopt the convention:
• L is a graded Lie algebra such that L — {Lj}j>i and
each Li is finite dimensional. ^ ' '
Recall from the start of §35 that the depth of the universal enveloping algebra,
UL, is the least integer m (or oo) such that Ex.t™L(k,UL) ? 0. Here we shall
slightly abuse language with the
Definition The depth of L is the depth of its universal enveloping algebra,
UL.
In §35 (Corollary to Theorem 35.10) we showed that if A" is a simply connected
topological space and if each H((QX) is finite dimensional then
depth Lx < cat0 X ,
where Lx is the homotopy Lie algebra of X. Thus spaces of finite rational
category have homotopy Lie algebras of finite depth.
Here we study graded Lie algebras L of finite depth m and show that
• The sum of all the solvable ideals in L is finite dimensional.
• UL is right noetherian if and only if L is finite dimensional.
• There are at most m linearly elements Xi in Leven such that ad x\ is locally
nilpotent.
As just observed these results apply to the homotopy Lie algebra of a space of
finite rational category. The reader should also note that the third result is a
considerable strengthening of Theorem 31.16.
This section is organized into the following topics:
(a) Depth and grade.
(b) Solvable Lie algebras and the radical.
(c) Noetherian enveloping algebras.
(d) Locally nilpotent elements.
(e) Examples.
The Rational Dichotomy 493
(a) Depth and grade.
Proposition 36.2
(i) If L is the direct sum of ideals I and J then
depth L = depth / + depth J .
(ii) If L is the infinite direct sum of non-zero ideals then depth L = oo.
proof: (i) Because of C6.1) we may identify Ext^L (k, UL) with Tor^1(k,UL*)*,
? > 0 (Lemma 34.3(iii)). Thus depth L is the least integer m such that Tor^A, ULS)
? 0. On the other hand, if P« and Q« are respectively VI- and UJ-iree resolu-
tions of k then P, ® Q, is a VI ® U J-free resolution of A. Since UL = UI®UJ
this gives
= H((P.®Q.)®UL(UIi®UJt))
= H (P. ®ui UI*) ® //(Q, ®i/j t/J»)
Assertion (i) follows.
(ii) Here there is no ? G UL such that (UL)+ ¦ a = 0 and so ExtyL (A, UL)
= H.omm(k,UL) = 0. Thus depthL > 1. But then for each ? > 1 we may
write L as the direct sum of ? ideals L(i), each of which is itself an infinite direct
?
sum. Thus by (i), depth L = ? depth L(i) > n. Hence depth L = oo. ?
Next, recall from Lemma 34.3(i) that if / C L is an ideal then ?????(%:, Jk) is
naturally a right ?/L//-module.
Proposition 36.3 Let I C L be an ideal,
(i) depth/ < depthL.
(ii) If a-UL/1 is finite dimensional for all a G Tor^I(k,k), q < depthL,
then depth L/I < depth L.
(Hi) If the hypothesis of (ii) holds and the Lie algebras L/I is finitely generated
then
depth / + depth L/I — depth L.
proof: (i) The Hochschild-Serre spectral sequence (§34(b)) converges from
??'9 = E^tpUL/I(k,E-KtqUI{k,UL)) to Extj^A-, UL). Since UL is UI-ivee
494 36 Lie algebras of finite depth
it follows that Ext^jfc.t/L) = 0, q < depth/. Hence ExtrUL(Jk,UL) = 0,
r < depth I and depth L > depth I.
(ii) Let m = depthL. We show first that Ext™L(k,UL/I) ? 0. In
fact, filter UL by the ?/L-modules Fp = UL-(UI)>p. Use the duality of
Ext2rL(Jb,i/L) and Tor^jfc, (UL)*) to deduce that for 0 ? a G Ext™L(Jfc, UL)
there is a maximum ? such that ? is in the image of Ext™L(k,Fp). It follows
that a maps to a non-zero element in Ext™L (k, Fp/Fp+1). But Fp/Fp+1 is
just i/L// <g> (UI)P as a t/L-module, and so Ext%L(k, UL/I) ? 0.
Next observe that the Remark in §34(b) identifies the u^-term in the Hochschild
Serre spectral sequence for Ext™L(k,UL/I) as
E™ = ExtpUL/I(ToT%I{jk,Jk),UL/l) .
Thus, for some ? + q = m, this term is non-zero and so (cf §35)
gradec/t// (TorJ^Jfc, Jfc)) < ? < depth L .
It is thus sufficient to prove that
depth L/I < gradeUL/J(M)
for any non-zero L//-module M for which ? · UL/I is finite dimensional, a G M.
Write M as the increasing union of sub L//-modules M(i) C M(i+ 1), i > 0,
with L/I acting trivially on each M(i)/M(i — 1). As in §20(b) there is a UL/1-
free resolution P, of M such that P« is the increasing union of free resolutions
P,(z) of M(i), and also P»(z)/P,(z - 1) is a free resolution of M(i)/M(i - 1).
Suppose now that Extp/L/j(k, UL/I) = 0. If ? G uomUL/j (Pp, UL/I) is a co-
cycle vanishing on Pp(i—1) then the induced cocycle in Horrim/j (Pp(i)/Pp(i — 1) ,
UL/I) is a coboundary by hypothesis. Thus for some b G HomUL/j (Pp_i, UL/I)
vanishing on Pp_i(z —1) we have: a — db vanishes on Pp(i). This argument shows
that ? itself is a coboundary and hence that ExtpJL,I(M,UL/I) = 0. Thus
gradeULjj(M) > depth L/I, as desired.
(iii) Recall that the Hochschild-Serre spectral sequence (§34(b)) con-
verges from Ext?rL// (Jc.Ext^Jc, UL)) to Ext^9(&, UL). The duality observa-
tions in §34(b) identify the E2-tevm with the dual of Tor^L// (k, Tot (k, (i/L)"))
and this coincidences with Tor^L// (k, (E/L//L) ® TorJ" (Jfc, ([//)'), by Propo-
sition 34.11, provided that q < depth L.
Since some ?1?'9 ? 0 for ? + q — depth L we may conclude that depth L >
depth L/I + depth /. Suppose inequality held and put r = depth L/I and s =
depth/. Then E?s ? 0 and ??+i's~i+1 = 0 = jE?-Ji'+j-1. This would imply
?oes 7e 0 contradicting depth L>r + s. ?
The Rational Dichotomy 495
(b) Solvable Lie algebras and the radical.
A graded Lie algebra L is called solvable if some ?/?+1) = 0, where L(n) is the
ideal defined inductively by
L<°> = L and Hn+^ = [tin\ L™] , ? > 0 .
The solvlength of L is the largest ? such that l/n) ? 0. Recall that we restrict
attention to those L satisfying L = {Lj}i>i and with each L{ finite dimensional.
Theorem 36.4 The graded Lie algebra L is solvable and of finite depth if and
only if L is finite dimensional. In this case EyLt*UL{k,UL) is one dimensional,
and
depth L — dim Leven .
proof: Suppose L is solvable and has finite depth. The ideal [L, L] has finite
depth (Proposition 36.3(i)). and so by induction on solvlength, [L, L] is finite
dimensional. In particular, for some k, L>k is an abelian ideal, also of finite
depth. Write L>* = @JkxQ and note that each kxa is an ideal in L>*. Since
a
L>k has finite depth it cannot be an infinite direct sum. (Proposition 36.2(ii))
and hence L>* is finite dimensional. Thus so is L.
Conversely, suppose L is finite dimensional. Since L — L>i, L is trivially
solvable. Moreover, a non-zero element ? in L of maximal degree is central. Set
kx = I and put
1 if deg ? is even
0 if deg ? is odd.
Since UI is either the exterior or polynomial algebra on kx, a simple direct
calculation gives
Extpj(k, UI) = 0 and dim Ext^Jk, UI) = 1 .
Thus Proposition 34.7 asserts that there are isomorphisms of ?/L//-modules,
ULI I iiq = e
0 otherwise.
In particular the Hochschild-Serre spectral sequence collapses at Eo, and
ExtrUL(k,ULJ?Extru-L'/J(k,ULII) .
It follows by induction on dim L that Ext*UL{k, UL) is one dimensional and that
depth L — dim LeVen· n
Definition The radical of a graded Lie algebra L is the sum of all the solvable
ideals of L.
496 36 Lie algebras of finite depth
Theorem 36.5 [54] If L satisfying C6.1) has finite depth then its radical, R,
is finite dimensional and dimi?even < depth L.
proof: Every solvable ideal I C L satisfies dim/even < depth L, by Theorem
36.4. Choose / so that dim 7even is maximized. For any solvable ideal J, I + J
is solvable, and hence JeVen C /even· It follows that i?even = /even and so. for
some k, R>k is concentrated in odd degrees. Thus R>k is abelian and R itself
is solvable.
Now Theorem 36.4 asserts that Ext^fi(ic, UR) is one-dimensional and con-
centrated in * = dimi?even· It follows that the isomorphism Extyfi(.fc, f/L) =
Ext*UR(k, UR)®UL/R identifies the left hand side with UL/R as a L/R-modu\e.
Thus the ?2-term of the Hochschild-Serre spectral sequence converging from
ExtUL/R (k,ExtgUR(k,UL)) to Extffj?(k,UL) is given by
,q = dimR
, otherwise.
In particular, the spectral sequence collapses at Eo, and depth L = depth L/R +
dimi?even. D
(c) Noetherian enveloping algebras.
A graded algebra A is right noetherian if any right A-submodule of A is finitely
generated. In this case any submodule of a finitely generated right module is
finitely generated.
Theorem 36.6 Suppose L is a graded Lie algebra satisfying C6.1). If L has
finite depth then
UL is right noetherian <==> dim L is finite.
Remark This Theorem was originally proved for homotopy Lie algebras of
spaces of finite category by Bogvard and Halperin [28].
proof: Suppose UL is right noetherian. Then any submodule of a finitely
generated right [/L-module is finitely generated. In particular k admits a UL~
free resolution P, such that each Pp is UL-free on a finite basis, and this implies
that each Tor^L(i:, k) is finite dimensional.
Next, note that any sub Lie algebra ? C L is also right noetherian. Indeed
(Corollary to Proposition 21.2) multiplication in UL defines an isomorphism
UE ® V ^> UL for some subspace V C UL. If M C UE is any subspace then
M · UL = M · UE <S> V. It follows that a minimal set of generators for the UE
module M · UE is also a minimal set of generators for M · UL as a ?/L-module.
In particular this is finite and so any right ?/i?-submodule of UE is finitely
generated; i.e., UE is right noetherian.
The Rational Dichotomy 497
Consider the ideal / = L>n, some ? > 0. Since / is right noetherian each
Tor^/(Ik, Ik) is finite dimensional and hence Proposition 36.3(ii) asserts that
depth L > depth L/I. Since L/I is finite dimensional Theorem 36.5 asserts that
dim(L//)even < depth L. This holds for I = L>n, any n, and so Leven is finite
dimensional and, for large n, L>n is concentrated in odd degrees. Then L>n is
abelian and L is solvable, hence finite dimensional (Theorem 36.5).
Conversely, if dim L is finite write L = ? ? kx where / is an ideal and ? is
a non-zero element of minimal degree. By induction on dim L we may suppose
UI to be right noetherian. Use multiplication in UL to define an isomorphism
UI ® Ax A UL.
If dega; is odd then UI <g> Ax is generated as a U/-module by 1 and ? and
any sub ?/L-module is finitely generated even as a U /-module. Suppose dega;
is even. If M C UL is a right f/L-submodule define subspaces S(k) C UI as
follows: ? G S(k) if and only if axk + ? ???% e M f°r some elements Oj G UI.
i<k
Then S(k) is a right U /-submodule. Since S(k) C S(k + 1) C · · · it follows
that for some n, S(n) = \J S(k), and we can find a finite subset of |J S(k) which
k k
contains a set of generators for each S(k), k < n. As in the classical case of
polynomial algebras it is straightforward to use these to construct a finite set of
generators for M as a [/L-module. Thus UL is right noetherian. ?
(d) Locally nilpotent elements.
An element ? G L is locally nilpotent or an Engel element (cf. introduction to
§31) if for all y e.L there is an n(y) for which (adx)n^y = 0.
Theorem 36.8 A graded Lie algebra L satisfying C6.1) and of depth m con-
tains at most m linearly independent Engel elements of even degree.
proof: We show that if L = / ? li, with / an ideal and ? a non-zero Engel
element of even degree, then depth / < depth L. (The theorem follows from this
by an obvious argument.)
To establish this assertion note first that since ad a; is locally nilpotent, x
acts locally nilpotently in each Tor^7(k,k), as follows directly from Example 2,
§34(a). Thus Proposition 36.3(iii) asserts that
depth / + depth kx = depth L
and the conclusion follows from depth kx = 1 (because ? has even degree). ?
(e) Examples.
Recall that graded Lie algebras L satisfy L = L>\ and each Lj is finite dimen-
sional.
498 36 Lie algebras of finite depth
Example 1 Depth = 0.
A graded Lie algebra L has depth0 if and only if HomUL(k,UL) ? 0; i.e..,
if and only if ? · UL+ = 0 for some non-zero ? G UL. It follows at once from
the Poincare Birkoff Witt theorem 21.1 that this occurs if and only if L is finite
dimensional and concentrated in odd degrees. D
Example 2 Free products have depth 1.
Let ? and L be graded Lie algebras and recall the free product EUL defined
in §21(c). We shall show that depth ? II L - 1.
Indeed, choose free resolutions of the form
A V{2) ®UEA V{1) ®UEAuE->R, and
? W{2) ® ?/? A W{1) ®UL^UL—>k.
Then as described in §21(c) there is a U(EU L)-free resolution of k of the form
[VB) © WB)] ® U(E II L) A [V(l) © W(l)\ ® U(E II L) A U(E II L) -» Jfc
in which the restriction of d to V(i) and W(i) is given by the resolutions above.
Choose non-zero elements ? G ? and y G L and define a U(E U L)-linear
map / : [V(l) ® W(l)] ® U(E U L) —> U(E U L) by f(v) = (dv)-yx and
f(w) = (dw) -xy. Trivial calculations show that / ? d = 0 and that / is not a
coboundary. ?
Example 3 XV Y.
Let X and Y be simply connected spaces with rational homotopy of finite type.
In Example 2 of §24(f) we observed that LXvy = LxULy. Thus depth Lxvy =
1.
On the other hand, cato(X VK) = max(cat0 X, cat0 Y) as follows from the
remarks at the start of §27. Thus the difference cato-depth can be arbitrarily
large. ?
Example 4 Products.
If ? and L are graded Lie algebras then
depth (? ? L) = depth ? + depth L
as observed in Proposition 36.2. Since Lxxy = Lx®Ly we have that depth Lxxy
= depth Lx + depth Ly in analogy with cato(^ ? Y) = cat0 X + cat0 Y (Theo-
rem 30.2) D
Example 5 X = S\ V 563 U[a[a,6]w]w ?>8·
This CW complex was first discussed in Example 2, §13(d) and subsequently
in Example 4, §24(f) and in Example 3, §33(c). In §33(c) we showed that the
homotopy fibre of the retraction X —> S% was rationally S$ V S5, and that the
fibre inclusion ? : S% V 55 —> X restricted to 55 represented [a, b]w-
The Rational Dichotomy 499
Recall that Lx is the rational homotopy Lie algebra of X. If ?, ? G (LxH
correspond to a,b G ??3(?) then it follows easily that Lx = L(a,/3)/[a, [a,/3]f.
As an immediate consequence we see that ? is an Engel element in Lx.
Write Lx = ha® /, where I = k? ? {Lx)>r The argument proving The-
orem 36.8 shows that depth/ < depthL. Since also depth/ > 0 (Example 1,
above) we have depth Lx > 2. On the other hand, X is a 2-cone and so cat A' < 2
(Theorem 27.10). Since depth Lx < cat0X (Theorem 35.10) we conclude
depth Lx = cat0 X = cat X = 2 . D
Example 6 CP°° /CPn.
Because the inclusion i : CPn —> CP°° induces the surjection Ax —> Ax/xn+1
in cohomology (deg a: = 2), the cohomology algebra of CP°° /CPn is just Q ©
xn+1 -Ax. Moreover, CP°°/CPn is 2n+1 connected and so ?2?+2(€?°°/???) =
H2n+2{CPO°l<CPn) = ?. This defines a continuous map / : CP°°/CPn —>
K(Z, 2n + 2). Let g : F —» CP°° /CPn be the homotopy fibre.
Next, observe that a Sullivan representative for i is also a Sullivan representa-
tive for the surjection Ax —> Ax/xn+l, (dega; = 2). Use Lemma 13.3 and 13.4
to deduce that A = k @ xn+1Ax is a commutative model for CP°°/CPn.
Extend the inclusion Axn+1 —> A to a minimal Sullivan model ? : (Axn+1 <8s
AV,d) A (A,0). This is a Sullivan model for CP°°/CPn and the inclusion
of Axn+1 is a Sullivan representative for /. Thus the quotient Sullivan algebra
(AV,d) is a minimal Sullivan model for F. Moreover since A is Aa:n+1-free on
the basis l,xn+2,... ,x2n+1 it follows that ? induces a quasi-isomorphism
2n+l
? : (? V, d) -=> Jfc ? 0 kxl -
i=n+2
Thus F is a formal space in which cup-products vanish and hence F has the
2n+l
rational homotopy type of V ^2l (Theorem 24.5).
i=n+2
Let L be the rational homotopy Lie algebra of CP°° /CPn. The discussion
above establishes the short exact sequence
0 —-> L(qi ,..., q„) —>· L —>· Q? —>· 0
with deg/3 = 2n + 1 and dega, = 2n + 2z + 1. Now Proposition 36.3(iii) asserts
that
depth L = depth Q? + depth L(qi ,..., ??) = 1 . D
Example 7 eo(A:) = 2; cato(A') = depth Lx = 3.
Consider the commutative rational cochain algebra (^4, d) = (?(?, b, {xn}n>i)/
I, d) with deg? = deg b = 2, degxn = 3n — 1, / the ideal generated by a2, b2 and
A-3({xn}n>i), and dx\ = 0 and dxn+\ = abxn. This is a commutative model for
500 36 Lie algebras of finite depth
some rational topological space X (§17). The inclusion A(a,b)/ar,b'2 —> (A,d)
defines a fibration Xq —> Sq ? Sq whose homotopy fibre is the infinite wedge
F = V S«" (same argument as in Example 6).
n>l
Consider the inclusion Sq V Sq —> Sq ? Sq and pull this fibration back to
a fibration Y —> Sq V Sq. A commutative model for Y is just ?(?. 6)/?-2?, b
®A(a,b)/a2,b2 A (same argument again), and this is just the tensor product H(SqV
Sq) ® H(F). It follows that Y ~q (S? ? Sq) ? F. Since S^vS^—iS^x SQ is
surjective in homotopy this implies that Lx is the direct sum of the ideals Lp
and ?s?xs?·
Now Proposition 36.2(i) asserts that
depth Lx = depth LF + depth L5? + depth L5? = 3 .
On the other hand, X has a commutative model with product length 3 and so
Theorem 29.1 states that cat AT < clA < 3. Since depth X < cat AT we have
depth X
= cat A = c\X = 3. Finally, if (AV, d) is a minimal Sullivan model for X
then any cocycle of wordlength > 3 in AV will map to such a cocycle in A, and
these are all coboundaries. It follows that e(A") < 2. If e(X) = 1 then cat A" = 1
(Theorem 28.5(ii)). Thus e(X) = 2. D
Example 8 L = Der>o Lv, where V is a finite dimensional vector space of
dimension at least 3.
Write V = ]kv® W where ? G Veven unless Feven = 0. Then there are infinitely
many linearly independent elements ? in L(W)even and each one determines
?? G DerLy by ??(?) = ? and 6X(W) = 0. It is straightforward to verify that
each ?? is an Engel element in L, and so depth L = oc. ?
Exercises
1. Let A" be a 1-connected finite CW complex and let Y be an r-connected
hyperbolic space such that dim X + 1 < r. Prove that the space of continuous
maps Map(A", Y), endowed with the compact-open topology, is a 1-connected
space. Prove that the evaluation map, M&p(X,Y) —> X, f ?—> f(xo), is a
fibration which admits a section. We denote by Mapt(X,Y) the fibre of this
fibration. Prove that depth Mapt(X, V) = depth Map(X, Y) — oc, so that
catMap(X,y) = oc.
2. Prove that the radical of the graded Lie algebra L = L(o, b)/[a[a, b]], with
dego = 2 = degb is trivial.
?
3. Let / : f\ S3 —>¦ S3N be the natural pinch map. Convert the projection
2=1
S3VS3N —> S3N into a fibration ? —>· S3N and consider the pull back fibration
?
X —> ?[ S3 along /. Compute the radical of ?*(??)
?=?
37 Cell Attachments
In this section the ground ring is Q except that in the first topic it is any com-
mutative ring.
Consider a cellular map
/ : Sn —> X
between simply connected CW complexes (recall from Theorem 1.2 that any
continuous map is homotopic to a cellular map). The first objective of this
section is to study the relationship between the rational homotopy Lie algebra of
X and of the CW complex Y = XUf Dn+1 obtained by attaching an (n + l)-cell
to X along /. We shall assume / is not a rational homotopy equivalence, so that
Y is not a rational point.
Convert the inclusion i '¦ X —>¦ Y into the fibration q : ? ? ? MY —>¦ Y, as
defined in §2(c). The fibre of P, F = ? ???? is the homotopy fibre of i, and the
holonomy action of Q.Y on F is simply right multiplication in PY. The inclusion
oi X xy PY inlxy MY is homotopic to the projection ? : X xy PY —>¦ X,
which is an fiy-fibration — the holonomy fibration for q. Note that because /
is null homotopic in Y it lifts to a map g : Sn —>¦ ? ? ? PY. Thus we have the
commutative diagram
XxY PY = F
Y = XUf Dn+1.
Let [5n] denote the fundamental class in Hn(Sn) (any coefficients). Our first
step is to prove
• H+(F) is the free ?'.(??)-module on the element Hn(g)[Sn].
We use this to give several equivalent conditions for ?, (i) <g> Q to be surjective, in
which case there is an explicit formula connecting the Hubert series for ULx =
Ht(QX;<Q) and ULY = H*(UY;Q).
Next we consider the case where X is a wedge of spheres and we attach a
family of cells: Y = ( \/Sa ) U/ ( [J Dni+l ), via a map / = {/,· : 5n; —»¦ X).
For these spherical 2-cones we derive the explicit formula for the Hubert series
of ULy originally established by Anick [6].
In [140] Serre posed the question: If Y is a simply connected finite CW com-
plex is the Hubert series of ULy rational? The original negative answer was
given by Anick [6]. Here we construct an example due to Lofwall and Roos.
502 37 Cell Attachments
It is a finite spherical 2-cone with the additional property that Ly contains an
infinite dimensional abelian Lie algebra (although it cannot contain an infinite
dimensional abelian ideal by Theorem 36.5).
This section is organized into the following topics:
(a) The homology of the homotopy fibre, ? ?? PY.
(b) Whitehead products and G-fibrations.
(c) Inert elements.
(d) The homotopy Lie algebra of a spherical 2-cone.
(e) Presentations of graded Lie algebras.
(f) The Lofwall-Roos example.
(a) The homology of the homotopy fibre, ? ?? PY.
The path space fibration PY —> Y restricts to the holonomy fibration X xY
PY —> X. In Proposition 8.4 we constructed a cellular model for C*(X ????)
of the form
(C ® C. (??), d) A C. (? ? ? PY) ,
where each Ck was free on the ft-cells of X. A slight modification of the con-
struction extends this to a cellular model of the form
This induces a quasi-isomorphism
) A C»(PY,X xY PY)
of C*(O.Y)-modu\es. The connecting homomorphism is an isomorphism of degree
-1 of H*(UY)-modu\es from H» (PY, ? ? ? ?? ) to H+{X ?? ??) and it follows
that H+(X ?? PY) is the free H*(UY)-modu\e on a single class of degree n.
Moreover, it is immediate from the construction that this class is H*(g)[Sn].
This establishes
Proposition 37.1 H+(X ?? PY) is the free H*(QY)-module on the single
basis element ?*(g)[Sn]. D
(b) Whitehead products and G—fibrations.
Let ? : ? —> ? be a G-fibration with fibre G at the basepoint * C B, where G
is any topological monoid. Given continuous maps ? : En+1,*) —> (B,*) and
b : Em,*) —> (E,*) we construct a continuous map
c: En+m,*)—>(?,*)
The Rational Dichotomy 503
as follows. Lift ? to ? : (?>?+1,5?,*) —> (E,G,*) and regard b as a map
(Dm,Sm-1) —»- (?,*). Then set
? b(x)-a(y) ,(x,y)€DmxSn
c{x, y) - j a^ ( ^ ^ e 5m_! ? ?)n+1
Now a, 6, e represent classes ? € ???+?(?), /3 G nm(E) and 7 € 7rn+m(jE),
and the restriction <9a : 5n —>¦ G of o represents the image «9,? of ? under
the connecting homomorphism <9, : ?,(?) —> ?»-?(?). Finally, recall that the
action of G on ? determines an action of H»{G) on H*{F) (§8).
Proposition 37.2 ([S-T]) With the notation above,
1»' and hur-y = hur ? · hur d*a .
proof: The first assertion is immediate from the definition of the Whitehead
product (§13(e)). For the second, observe that c factors over the surjection
d(DmxDn+1) —>· (SmxSn)UDm+1 todefinec : (SmxSn)UDm+1 —>· E. More-
over, hur-y = H*(c) [d(Dm ? Dn+1)] = H»{c) ([Sm] ® [5n]) = hur?-hura,
where [ ] denotes fundamental class. D
(c) Inert elements.
We maintain the notation established at the start of this section. Recall also
that Lz = ?, (Q.Z) ® Q denotes the homotopy Lie algebra of a simply connected
space Z, and that the connecting homomorphism of the path space fibration is
an isomorphism ?,+1(?) <g> Q -—> Lz-
In particular, let ? € (Lf)u-i and ? € (?,?)?_! correspond to [g] and to [/].
Then ?,(??)? = ? and so ?,(??)? = 0.
Theorem 37.3 The following conditions on [/] are equivalent:
(i) F has the rational homotopy type of a wedge of at least two spheres,
(ii) ?, (??) : Lx —> Ly is surjective.
(in) ?,(??) is surjective, and its kernel is the Lie ideal I generated by ?.
(iv) The ideal I generated by ? is a free Lie algebra, and the quotient I/[I, I]
is a free ULx/I module on the single generator, ?.
Remark An element ? in a graded Lie algebra L is called inert if the Lie ideal
/ it generates is a free Lie algebra and if also //[/, /] is a free UL/I module on
the single generator a. Thus Theorem 37.2 asserts that Lx —> Ly is surjective
if and only if w is inert.
504 37 Cell Attachments
proof of Theorem 37.3: Recall that we use rational coefficients throughout,
so that #,(-) = H»{ ;Q). First observe that (i) ? (ii). Indeed, if (i) holds then
Lp is a free Lie algebra on at least two generators (Theorem 24.5) and hence has
no central elements. But in §28 (Example 2 combined with Proposition 28.7)
we showed that elements in the kernel of k*{F) —> ?*(?) correspond to central
elements in Lp. Thus Lp —> Lx is injective and Lx —> Ly is surjective.
Conversely, if Lx —> Ly is surjective then it follows from Proposition 37.2
that the subspace hur[g] · ULy is in the image of the Hurewicz homomorphism,
hur : 7r,(F) 0Q-> H.(F). Since hur[g] -ULY = H+(F) (Proposition 37.1) it
follows that Im hur = H+(F). Now Theorem 24.5 asserts that F has the rational
homotopy type of a wedge of spheres (at least two because Y is non trivial).
Next observe that if (i) and (ii) hold then ?,(??) : Lp -^ kein*(Qi). Since
/ C ker7r,(Sz) (because ?,(??)? = 0) it is, in this case, a sub Lie algebra of
a free Lie algebra. By the Corollary to Proposition 21.4 / itself is a free Lie
algebra. This shows that under any of the hypotheses (i)-(iv), / itself is a free
Lie algebra.
Factor ?,(??) to define a morphism
? : Lx/I —y Ly
and note that / C n*(Qp)Lp (= ker^(fiz))· It is immediate from the long exact
homotopy sequence that;
? is an isomorphism in degrees < r <==? ?,(??) : (Lp)<r -^> I<r . C7.4)
Moreover, since / is a free Lie algebra we may choose a wedge of spheres
5 = y Sha+1 such that Ls = I, and since / C Imn*(Qp) we may construct
a continuous map
h:\JSk*+1 —>F
a
such that ?, (??) ? ?, (?/i) is the inclusion of / in Lx.
The main step in the proof of the theorem is
Lemma 37.5 If any of the conditions (i)-(iv) of Theorem 37.2 hold then ? is
an isomorphism.
proof: Suppose ? is an isomorphism in degrees < r. Then C7.4) shows that
?.(?) : 7r<rE)®Q A K<r(F)®Q. Extend h to h' : S' = SvV 5[+1 V\/ 5J+2 so
* i
that ?»(/?') is a rational isomorphism in degrees < r + 1 and rationally surjective
in degree r + 2. Then (proof of Theorem 8.6 or via an easy argument using
Theorem 7.5) H»(h!) is an isomorphism in degrees < r + 1.
Let ? e 7rr+i(F) <g> Q correspond to ? e (Lf)»·· Then hur ? = hur[g] -? for
some ? e (ULy)r+i-n. Since ? is an isomorphism in this degree we can write
? = ??' for some ff e ULX/I. Thus Proposition 37.2 asserts that hur ? = hur ?'
The Rational Dichotomy 505
where ?»(?)/3' is a linear combination of iterated Whitehead products starting
with [/]. However, the correspondence ?»(A')<g>Q = (L.y)»_! converts Whitehead
products to Lie brackets (Proposition 16.11). Thus if a' € (Lp)r corresponds to
?' then ?»(??)?' € /. Moreover, the image of ?»(??)?' in //[/,/] is just ? ·?'.
On the other hand, since ? — ?'?. ker hur and since ??+? (?/) <g> Q and Hr+i (h1)
are isomorphisms it follows that ? — ?' = ??+?(/?'O, where 7 e ??+?E') <g> Q is
in the kernel of the Hurewicz homomorphism for 5'. Hence ? — ?' = ??+?(?O,
where 7 € ??+1 (,5)<8>Q corresponds to an element 7 € [L5, L5]. Thus ??,(??)(? —
We are ready to show that ??+? is an isomorphism. Indeed, ?, (??)? =
?»(??)?' + ?»(??)(? — ?') € /, as just shown, and so K:,(Clp)(Lp)r = Ir. On
the other hand if (i), (ii) or (iii) hold then ?,(??) ". Lp —>¦ Lx is automatically
injective (because (i) => (ii)). Suppose (iv) holds and that ?.(??)? = 0. As
just shown ?»(??)(?' — ?) € [/,/] and ?,(??)?' represents ?-?' in //[/,/].
Since ULx/I acts freely on ? in //[/,/] it follows that & — 0 and so hur ? =
hurlgj-??' = 0. This implies (as above) that ? € nr+i(h) (??+1E) <g> Q) and
hence that ? € ?,»(?/?)?,5. Since ?»(??) is injective in this subspace, ? = 0; i.e.
?»(??) : (LF)r+i —> (Lx)r+1 is injective. D
We now return to the proof of Theorem 32.3. The Lemma asserts that if
(ii) holds then ? is an isomorphism. This implies at once that (ii) => (iii).
Moreover since ? is an isomorphism if (iv) holds then in this case ?. (??) :
Lp —i- I and hence ?,(??,) : Ls —>¦ Lp is an isomorphism, and h is a rational
homotopy equivalence. Thus (iv) =$¦ (i). Finally if (iii) holds then again h :
5 —>¦ F is a rational homotopy equivalence. This defines a degree 1 isomorphism
Ls/[Ls,Ls] -=> H+(F). Now Ls = I and it follows from Proposition 37.2 that
the action of Lx/I in //[/, /] corresponds to the action of Lx/I in H+(F). Since
the latter is a free ULx/I module on a single generator (Proposition 37.1) so is
the former. Thus (iii) => (iv). D
(d) The homotopy Lie algebra of a spherical 2-cone.
Consider a continuous map
f:Z = V5m"+1 —>X = VSna+1
? a
between two simply connected wedges of spheres with rational homology of finite
type; and write Y = X U/ CZ. Then (Theorem 24.5) Y has a free Lie model of
the form (Lv<sw,d) in which:
sV = H+(X), dV = 0 and W = H+{Z) and d:W—>lv-
Assign a bigrading to this differential graded Lie algebra by setting Vp = VQ^P
and Wq = Wi,9_i. Then d has bidegree (—1,0). In particular the homotopy Lie
00
algebra Ly = H (Lv&w,d) inherits a bidegree: LY = 0(Ly){ ». This exhibits
i=0
506 37 Cell Attachments
Ly as the direct sum
LY = L ? /
of the sub Lie algebra L = (LyH,, and the ideal / = (Ly)+i*.
Proposition 37.6 With the hypotheses above, I is a free graded Lie algebra.
proof: Denote h\/®w simply by L. Then o^Lx,,) is an ideal in Lo., and so
? = L+,, © d(Li,,) is an ideal, in L with quotient h/E = (L,0).
Now apply the cochain construction of §23 to obtain a relative Sullivan algebra
Cm(L,0) —>· Cm(JL,d) with Sullivan fibre C"(E,d). Recall that C"(L) = A(sL)%
which we bigrade by setting (sLp,,)9 = [(sL)lt]p+1'9. Thus the differential in
C*(L) has bidegree A,0).
Now let (E',d) C (E,d) be the sub Lie algebra given by
@ if ? = 0
kerd if ? = 1
Ep ifp>2.
The inclusion (E',d) —>¦ (E,d) is a quasi-isomorphism and so (Proposition 22.5)
C*(E,d) -=> C*(E',d). In particular it follows that:
H+ (C*(E,d)) is concentrated in bidegrees (p,q) with p>2. C7.7)
On the other hand, H+ (C*(L, d)) ? ? ((sV ? sTV)8,d), by Proposition 22.8.
Hence H+ (C*(L,d)) is concentrated in bidegrees (p,q) with ? = 1,2. It follows
that there is a quasi-isomorphism C*(L, d) -^ (^4,d) of bigraded commutative
cochain algebras in which A = Q © .41'* © /I2'*.
Finally, we can extend C*(L,0) to the acyclic relative Sullivan algebra
C* (L, (UL)^) by dualizing the construction of §23(c). Then we have quasi-
isomorphisms
C*{E) A C*(L) ®c*(L) C* (L, (UL)*) -»¦ ^ ®C.(L) C* (L, (UL)*) .
Now v4<8>c-(L)C* (L, (ULf) = v4®(?/L)8 is concentrated in bidegrees (p,*) with
? < 2, because (L)9 is concentrated in bidegrees @, *). It follows that H (C*(E))
is concentrated in bidegrees (p, *) with ? < 2. Comparing this with C7.7) we
see that H+ (C*(E,d)) is concentrated in bidegrees B,*).
This implies that there is a quasi-isomorphism C*(E, d) -=» (k ? if2'*, ?) with
multiplication in H2'* trivial. It follows that / = H(E,d) ^ H(CC(E,d)) =
L(s-1ii2'*) is a free graded Lie algebra (use Theorem 22.9). D
Recall from §3(e) that the Hubert series for a graded vector space V>0 is the
oo
formal power series V(z) = J^dimVnzn. Similarly, if H = H^° then H{z) =
?
The Rational Dichotomy 507
Proposition 37.8 (Anick [6]) With the notation preceding Proposition 37.6,
ULY(z)-* = A + z)UL(z)-1 -(z- H+{Z){z) + H+{X){z)) .
Corollary If X and ? are finite wedges then ULy(z) is rational if and only if
UL(z) is rational.
proof of Proposition 37.8: If Co <— C\ <— ·¦· <— Cn is a finite dimen-
sional chain complex then ?(—l)p dim HP(C) = ?(—l)pdimCp, as follows by a
trivial calculation. Hence if Co,* <— Ci,, <— ··· <— Cn,« is a finite complex
of graded vector spaces of finite type with differential of bidegree (—1,0) then
?(-1)? dim Cp,q = ?(-1)? dimHp,,, q > 0. This implies that
Apply this (in the notation of the proof of Proposition 37.6) to the complex
(A ® (ULf) = A* ®UL to obtain
[A2>*(z) - ???'·(?) + ?2 ?0'* (?)] UL(z) = [s S Os) + ?2] ,
where / = L5. On the other hand since C*(L, d) -=> (A, d) it follows that H+(A)
is the dual of the homology of the complex
{sVf —»¦ {sWf .
Thus
= H2'*{A){z) - ???'·{?){?) + z2H°>*(A)(z)
= (sW)(z) -z(sV)(z) + z2
= H+{Z){z)-zH+{X){z) + z2 .
Finally, ULY(z) = UI(z)UL(z) and UI(z) = TS(z) = A - S(z))-1. A short
calculation completes the proof. ?
(e) Presentations of graded Lie algebras.
Let L = L>i be a graded Lie algebra. We may always write L = Ly /J where
J C [Ly ,Ly] is an ideal in the free graded Lie algebra Ly. In this case a basis
(vQ) of F is a minimal set of generators of L. Similarly the Lie bracket defines
a representation of Ly in J. Decompose J = R® J · ?/Ly. Then a basis (r/j) of
R is a minimal set of generators for the ideal J. The representation
L = h(vQ)/(r3)
is called a minimal presentation of L.
508 37 Cell Attachments
Proposition 37.9 With the notation above (s2 = double suspension),
(i) sV = Tor"L(Q,Q).
(ii) s2i?STor^(Q,Q).
proof: (i) Indeed V = U//[Ly,L;/] = L/[L,L] and so sV = Torf1"(Q,Q) by
Example 1, §34(a).
(ii) Consider the Hochschild-Serre spectral sequence converging from
TorJ^ (Q,Tor^J(Q,Q)) to Tor^v(Q,Q). Again by Example 1 of §34(a) we
may identify TorfJ(Q, Q) = s (J/[J,J]) with the representation of UL induced
by Lie bracket in J. It follows that
El, = Tor%L (Q,TorfJ(Q,Q)) ~ s (J/[J, J] ®UL Q) ~ sR .
On the other hand since Lv is free we have ???^? (Q, Q) = sV and Tor^v (Q> Q)
= 0 — cf. Proposition 21.4. Since Effi = Tor^ (Q, Q) = sV it follows that the
differential d2 in the spectral sequence satisfies
d2 : E2fl = To#L(Q, Q) A E2tl ^ sR . D
Again let L = L(uQ)/(r/j) be a minimal presentation of a graded Lie algebra
L = L>\. Then without loss of generality we may suppose the rp are integral
combinations of Lie brackets of the vQ. Put deguQ = nQ and degr/j = ???. Then
Lv is the homotopy Lie algebra of the space X = V 5na+1 and the r? define a
a
continuous map
/ : ? = \fSm0+1 —>X = VS"Q+1 ·
? a
As in §37(d) set Y = X U/ CZ. Then as described there the homotopy Lie
algebra Ly is the direct sum of a sub Lie algebra and an ideal /, and the sub
Lie algebra in question is exactly
L = L(vQ)/(r?) .
Thus we may reinterpret Proposition 37.9 as
H+ (X) = Torf1"(Q, Q) and sH+{Z) ^ Tor^L(Q, Q) .
In particular, L is finitely presented if there are only finitely many generator va
and finitely many relations rp and in this case Y = X U/ CZ is a finite CW
complex. Since L is a sub Lie algebra of Ly we deduce:
Theorem 37.10 Let L = L>, be a finitely presented Lie algebra. Then there
is a finite simply connected CW complex Y = X U/ CZ whose homotopy Lie
algebra Ly is the direct sum
Ly = L@I
The Rational Dichotomy 509
of L as a sub Lie algebra and an ideal I. Moreover I is free as a graded Lie
algebra and
ULY(z)-1 = A + z)UL(z)-1 -(z- H+(Z)(z) + H+{X){z)) .
(f) The Lofwall-Roos example.
Here we construct a finite CW complex Y, due to Lofwall and Roos [112],
such that the Hubert series ULy is irrational and also Ly contains an infinite
dimensional abelian Lie algebra.
To begin, note that the cohomology algebra H = H ((S'2 V S2) ? (S2 V S'2))
has the form [?(?, b)/a2, ab, b2] ® [A(x, y)/x2, xy, y2] where a,b,x,y are classes of
degree 2 corresponding to the four copies of S2. Put V = ? kvi with deg vi = 2
and define a relative Sullivan algebra
(H ® AV, d).
by setting dv2 - 0, dv3 = (b + y)vs + ax and dvi = (b + (-l)i+1j/)^'-i) i > 4-
Now the minimal Sullivan model for ? is the cochain algebra (AW,d) =
C* (LE,6)) <g> C* (L(x,j/)), whose differential is the purely quadratic operator
given by the Lie bracket. The quasi-isomorphism (AW,d) —> (-#,0) extends
to a wordlength preserving quasi-isomorphism of the form (AW <g> AV, D) —>
(H <g> AV, d). Thus it is also a morphism (AW <g> AV, D2) —> (H ® AV, d), where
D2 is the quadratic part of D. However, D = D? in AW, and the quotient
differential ~D in AV satisfies ~D = d = 0, so that ~D = ~D2 = 0. It follows that
(AW®AV,D2) -^(H®AV,d).
Let H^ (AW®AV, D-2) be the cohomology represented by cocyles of wordlength
p. Then H^(AW ® AV,D2) = H^(H ® AV,d) and a short calculation shows
that bases for H^(H ® AV,d) and H^(H ® AF,d) are given by.
H^ : a,b,x,y,V2
H^ : ax,ay,bx,by,av2,xv2,v\ .
On the other hand (Example 1, §23(a)) a graded Lie algebra L is determined
by the condition
C*(L) = (AW®AV,D2) .
Moreover, H^ = Ext^L(Q,Q) and H& = Ext^L(Q,Q) are dual to TorfL (Q, Q)
and Tor^L(Q,Q). Thus (Proposition 37.9) L is finitely presented with five gen-
erators in degree 1 and seven relations in degree 2. Let
510 37 Cell Attachments
be the corresponding CW complex described in Theorem 37.10. Then L is a sub
Lie algebra of Ly and
ULY{z)'1 = A + z)UL(z)-1 - (z - 7z3 + 5z2) .
Finally, since C*{L) = (AW ® AV,D3) we have (sL)» = W ? F with Lie
bracket dual to D-i- Since .?>2 = 0 in AV it follows that there is a short exact
sequence
0—> I —*L —>· L(a,b) ? L(x,j/) —>0
where / is an abelian ideal in L with basis U2,U3, · · · , and degu^ = A; — 1. In
particular
• Ly contains the infinite dimensional abelian sub Lie algebra ? Qufc.
k
• ULy1 is the irrational power series
Exercises
1. Let i : X —>· F = X U Dn+1. Prove that:
(a) If i : CP2 —>¦ CP3 denotes the natural inclusion then dimkerTr, (??) —
dim Coker ?,(??) = 1.
(b) If i : 53 V 53 —>· 53 ? 53 denotes the natural inclusion then ?, (fii) is
surjective.
(c) If i : Xe—>X V 5n, ? > 2, denotes the natural inclusion then ?, (??) is
not surjective and dim Coker ?,(??') — oo.
2. Let X be a simply connected topological space with rational homology of
finite type. Using Theorem 29.1, prove that if cat0 X = 2 then cl0 X = 2.
3. Let F be the homology fibre of the inclusion i : X c—> X U Dn+1 = Y and let
d : UY —> f be the inclusion {*} ? UY <^-> F xy PF. Prove that if H,d ? 0
then depth H, (QY) = 1.
38 Poincare Duality
In this section the ground ring k is an arbitrary field of characteristic zero.
Suppose A = {v4!}o<?<n is a finite dimensional commutative graded algebra
such that A0 = k, and suppose ? a is an element in the dual space (An)a. Define
bilinear maps ( , ) : An~p ? Ap —> Jk by
(a,b) =uA(a-b) , a € An~b , b e Ap .
Definition .4 is a Poincare duality algebra with fundamental class ? a if the
bilinear maps ( , ) are all non-degenerate scalar products. In this case ? is called
the formal dimension of A.
If M is a compact simply connected manifold then its cohomology algebra
H*{M) satisfies Poincare duality [68] and this is the principal condition that a
simply connected space must satisfy in order to have the rational homotopy type
of a compact manifold [18].
In this section we consider the rational homotopy theory of simply connected
topological spaces X whose cohomology satisfies Poincare duality. The main
results are:
• If X is rationally elliptic then H*(X) satisfies Poincare duality.
• If H*(X) satisfies Poincare duality then eo(X) = cato(-^)·
·¦ Suppose X satisfies Poincare duality with formal dimension ? and write
X = ? U/ Dn so that Hn(Dn,Sn~1) A Hn{X). Then the element ? ?
(Lx)n-2 corresponding to [/] € ??-?(?) is inert.
This section is organized into the following topics:
(a) Properties of Poincare duality.
(b) Elliptic spaces.
(c) LS category.
(d) Inert elements.
(a) Properties of Poincare duality.
Let A be a finite dimensional commutative graded algebra of the form k ?
{¦^!}?<?<? and suppose ? a G An. The bilinear maps ( , ) defined above deter-
mine linear maps ? : An~p —> {??? by
9(a)(b)=uA(a-b) = (a,b) .
Since (.<4p)tt = (As)~p, we may regard oasa linear map A —> A^ of degree —n.
512 38 Poincare Duality
Lemma 38.1
(i) A satisfies Poincare duality if and only if ? is an isomorphism.
(ii) If ? = L·® {Bl}i<i<m is a second finite dimensional commutative graded
algebra and if A <g> ? satisfies Poincare duality then so do A and B.
(Hi) If A is equipped with a filtration and the associated graded algebra satisfies
Poincare duality then so does A.
proof: (i) is by definition, (ii) follows from ??®? = #.4 ®?? and the fact that
a tensor product is an isomorphism if and only if both tensorands are. (iii) is an
obvious calculation. D
Next consider a commutative differential graded algebra (.4, d), and note that
(A*,dl·) is a chain complex.
Lemma 38.2
(i) If A is a Poincare duality algebra and with fundamental class ? ? € (???
and if Su a = 0, then H (A) is a Poincare duality algebra with fundamental
class [? a}·
(ii) Suppose ? € Aeven is a cocycle and define (A®Au,d) by du = ?. If H {A) is
a Poincare duality algebra, then H(A(&Au, d) is a Poincare duality algebra.
proof: (i) Because euA = 0, BAd = (~l)nSeA and ??(?) = ? (? a) is an
isomorphism.
(ii) This is a simple exercise left to the reader. D
(b) Elliptic spaces.
Proposition 38.3 If (AV, d) is an elliptic Sullivan algebra (introduction to
§32) then H(AV,d) is a Poincare duality algebra. Its formal dimension is ? =
X^degXj — X3(degy_; — 1), where (??) is a basis of Vodd and (yj) is a basis of
i ?
¦(/¦even
proof: Recall the odd spectral sequence defined in §32(b). Its first term is
(AV,da) with tk(I/even) = 0 and ??(???) c Veven. Proposition 32.4 asserts
that H(AV,da) is finite dimensional.
Choose r sufficiently large that each yj = d^j and extend (AV, da) to (AV <g>
AU,da) by assigning U the basis (uj) and setting daUj = j/J. Then (AV <g>
(\ 1 \
AU,da) -?» ®Ayj/uJ ®AVodd,do- and it follows from Lemma 38.2(ii)
\[j J /
that H(AV <g> AU, ??) satisfies Poincare duality. On the other hand, H(AV <g>
The Rational Dichotomy 513
AU,da) = ?(??,??) <8>A(ui - ??,... ,u, - ?,) and so Lemma 38.1(ii) asserts
that H(AV, da) satisfies Poincare duality.
Finally, Theorem 32.6 shows that ? = X^degZj — J2(degyj — 1) is the top
* i
degree in which both (AV,da) and (AV,d) have non-vanishing cohomology. Since
H(AV,da) is a Poincare duality algebra, Hn(AV,da) — k[z] and so this class
must survive through the spectral sequence. Thus if Ep is the p-th term of the
spectral sequence then (E%)* = ku\ and ?*?? - 0. By Lemma 38.2(i), each Ep
is a Poincare duality algebra. Hence the bigraded algebra associated to H(AV, d)
is a Poincare duality algebra and thus so is H(AV,d) (Lemma 38.1(iii)). ?
(c) LS category.
Theorem 38.4 Let X be a simply connected topological space and let (AV,d)
be a minimal simply connected Sullivan algebra such that H(X) and H(AV,d)
are Poincare duality algebras. Then
eo(X) = catoX and e(AV,d) = cat(AV,d) .
Corollary If X and (AV, d) are elliptic then eo(X) = cato.Y and e(AF, d) =
cat(AV,d).
proof of Theorem 38.4: Choose ? € (AV)& so that d*z = 0 and ? represents
a fundamental class of (AV,d). Define ? : (AV,d) —> ((AF)",^) by setting
??(?) = ?(? ? ?), ?, ? € AV. Then ? is a linear map of (AV,d)-modules and
?(?) is an isomorphism because H(AV, d) satisfies Poincare duality.
On the other hand in §29(h) we introduced the invariants meat and e for
(AV, c?)-modules (M,d) in terms of a semifree resolution of (M,d). In particu-
lar, these invariants coincide for quasi-isomorphic modules. Thus according to
Theorem 29.16,
e(AV,d) = e ((AVy,d*) = cat(AV,d) .
Finally, the assertion for X follows from this via the minimal Sullivan model
for X. D
(d) Inert elements.
Theorem 38.5 (Halperin-Lemaire [85]) Suppose X = ZU/Dn is ? simply con-
nected space such that H*(X) is a Poincare duality algebra of formal dimension
n, and Hn{Dn,Sn) -=> Hn{X). If the algebra H*(X) is not generated by a
single element, then the element a € (Lz)n-2 corresponding to [/] € 7rn_i(Z) is
inert.
proof: Let (AV,d) be a minimal Sullivan model for X and extend it to a
minimal relative Sullivan algebra (AV <g> AV, d) such that H[AV ® AV*, d) = Jfc.
514 38 Poincare Duality
Thus the linear part of d will be an isomorphism V -^> V, which we denote by
V ? > V.
Because X is simply connected, V = V^2 and d = 0 in V2. Thus V2 =
H2(AV,d). Write (AV)n = Jn ? ??, where ? is a cocycle representing a ba-
sis element of Hn(AV,d), and Jn D (Imc?)n. Poincare duality implies that
multiplication ?2 ? Hn~2 —> Hn is non-degenerate. It follows that there
is a subspace Jn~2 C (AF)" such that Jn~2 ? (kerc*)" = (AV)n~2 and
such that V2 · Jn~2 C Jn. Now extend these subspaces to a differential ideal
(J,d) C (AV,d) by setting Jk = 0, k < ?-2, Jn~l = (AVO1'1 and Jk = (AV)k,
k > n. Again by Poincare duality, ??"'(?7, d) = 0 and so it follows that
(AV, d) —> (AV/J, d) is a quasi-isomorphism.
Denote AV/J simply by A and denote the image of ? by ?. Then a Sullivan
representative for (A, d) —> (?/??, d) is also a Sullivan representative for the
inclusion i : ? —> X. Thus if we define (.4 ? ku,d) by setting du — ? and
u · A+ = 0 the composite (AV,d) -=» (A,d) —> (?? hu,d) is also equivalent to
a Sullivan representative for i.
Extend this composite to a quasi-isomorphism (AV <g> AW, d) -^» (? ? Jku, d)
from a relative Sullivan algebra (AV ® AW, d). Then Proposition 15.5 identifies
the quotient Sullivan algebra (AW, d) as a Sullivan model for the homotopy fibre
of i. Thus by Theorem 37.3 we have only to show that (AW, d) is a Sullivan
model of a wedge of spheres.
Now, as observed in Lemma 14.2, we have quasi-isomorphisms
(AW, d) ^ (AV <8) AW, d) ®?? (AV <g> AV, d)
= (AV <8) AW <8) AV, d)
¦=> ((A<5>]ku)®AV,d) .
Suppose now that the least integer r such that Vr ? 0 is odd. In this case
we shall construct a quasi-isomorphism (B,0) -=> ((^4 0 fcu) <&AV, d) with
B+ 'B+ = 0. By the Example of §12(c), (B,0) is a commutative model for
a wedge of spheres, and hence (AW, d) will be the Sullivan model for the wedge.
For this observe that Hr(AV,d) = Vr = Ar. Choose ? ? 0 in Ar and (by
Poincare duality) a cocycle ? € An~r such that vz = ?. Then A+ = Mv ? /,
where / is the differential ideal in A of elements a satisfying za — 0. Extend ? to
a basis ?, ??,..., Ok, · · · of AV with degOk < degufc+i and define ? : AV —i AV
by 6(vsa) = ^fj-a, a € ?(??,... ,vk,...).
Now dv = v. Suppose dvq e 7_(g) AF for 1 < q < k. Then dvk = ? <g> ? -I- w,
? € ?(?,.. .ojfc-i), w e I (g) AV, and d(vk - 0?) el® AV. JReplace vk by
vk — ?? and continue in this way to arrange that dvk € / <g> AF for all i < k.
Let -B+ c(i9 ku) (8> ? F consist of the elements of the form u <g> ? - ? <g> ??,
? € AF. Since ?« = ? and /? = 0 it follows that d(B+) = 0 and B+ · B+ = 0.
Since ii(yl <g> AF) = A it is immediate that the inclusion of ? = h 0 B+ in
AF is a quasi-isomorphism, as desired.
The Rational Dichotomy 515
We now deal with the general case. Let I be the least odd integer such that
Ve ? 0. We prove the theorem by induction on ? — ? dimV\ The case
0<i<i
? = 0 is the case above when the least positive integer r such that V ? 0 is
itself odd.
For the inductive step we may take r even and, as above, write A+ = hv ? /
where ? is a non zero element of degree r, ? is a cocycle such that vz = ? and
I = {ae A\az = 0}.
Now write V = kv ® V\. Since ? has odd degree H(AV <g> Av, d) also satisfies
Poincare duality, with formal dimension ? + r — 1 (Lemma 38.2(ii)). Moreover
we have quasi-isomorphisms
(AV, d) #- (?V <g> AC, d) -^ (A <g> ??, d) <^ (Ax, d)
where Ai C A <8> ?? is the subalgebra i: ? (/ (g) ??) ©?;(?®?). On the one hand
this implies that H(A,d) is a Poincare duality algebra with top cohomology
class represented by ? (g) v. On the other hand, it allows us to assume that the
differential in (A <8> AV, d) sends V\ into Af <g> AV^.
Since the theorem is true by the induction hypothesis for (AVi,<i) and since
?(??®?) = ?®? and (u®d)(Af) = 0 it follows that (B,d) = ([yli © J:(u ® ?)] <8>
AVi,<i) is a commutative model for a wedge of spheres.
Finally, since (??, d) -^> (yl <g> ??, c?) it follows that
([?? ? (Au <8> ??)] (8) AVx, d) -^((ie Au) <8> AV, d) .
This source algebra can be written as ? = B\ ® (u — ?® ?)???.
By construction d(u — ? ® v) = ? — ? = 0 and (u — ? (g) ?)?+ = 0. It follows
that (u — ? <g> ?)??? consists entirely of cocycles that multiply Z?+ to zero. Thus
since {B\, d) is a commutative model for a wedge of spheres so is (B, d), and the
theorem follows by induction. ?
Exercises
1. Let / : M —> ? be a continuous map between 1-connected n-dimensional
compact manifolds. Suppose that Hn(f;Q) ? 0. Show that cato(M) > cato(iV).
2. Let / : M —> ? be a smooth map between 1-connected compact manifolds.
Prove that if either
(a) / is a fibration whose cohomology Serre spectral sequence collapses at the
?2-term,
or else
(b) or else, / is a locally trivial bundle whose fibre F satisfies x(F) ? 0,
then cato M > cato N.
516 39 Seventeen Open Problems
39 Seventeen Open Problems
We close this monograph with seventeen of our favourite problems, all of which
remain open as this goes to press, and many of which are decades old. Other
compilations of problems can be found in [46] and [147].
1. Suppose F is a rationally elliptic space with non-zero Euler-Poincare charac-
teristic, and F —> ? —> ? is a Serre fibration of simply connected spaces. Does
the (rational) Serre spectral sequence always collapse at E-2 ?
A positive answer was conjectured by Halperin in 1976. The cohomology of
such spaces F has the form H = ?(??,... ,xn)/(fi, ¦ ¦ ¦ > /?), where xi,...,xn
have even degrees and f\,..., fn is a regular sequence in the polynomial algebra
?(??,..., xn). Thus an equivalent formulation of the problem is:
For graded algebras of the form ? is zero the only derivation of neg-
ative degree?
Positive answers to this question have been given in the cases that: all the
Xi have the same degree ([161]), ? < 2 ([146]), ? < 3 ([113]). if F is a homoge-
neous space ([141]), and if each polynomial fi is homogeneous with respect to
wordlength ([120]). In [132] it is shown that the answer is positive in the generic
case.
Essentially the same question has arisen in the context of deformation of sin-
gularities ([154], [36]) where attention is restricted to the case that fi = J?· for
some single polynomial /.
2. Suppose an r-torus acts continuously with finite isotropy groups on a closed
simply connected manifold M. Is it true that dimH*(M;Q) >2r?
The torus rank conjecture asserts that the answer to this question is positive.
It can be easily reformulated in terms of Sullivan models as follows:
Suppose a simply connected Sullivan algebra A(V, d) whose cohomol-
ogy satisfies Poincare duality occurs as the Sullivan fibre of a relative
Sullivan algebra of the form (?(??,..., xr) <8> AV, d) with deg ?? = 2,
1 < i < r. If ? (A(xi,..., xr) <g> AV) is finite dimensional, is it true
that dim H(AV, d)>2r?
The torus, rank conjecture has been established for homogeneous spaces ([83]),
for homology Kahler manifolds ([4]), for r < 3 ([5]) and for cohomologically
symplectic manifolds satisfying the hard Lefschetz theorem ([115]). It implies
that if L = L>i is an evenly graded finite dimensional Lie algebra over Q, then
dimExtUL(Q.~Q) >2dimcentreA').
3. If X is any finite simply connected CW complex and if ? > dimX is there a
rationally elliptic CW complex Y such that X ~q Yn, Yn the ?-skeleton ofY?
The Rational Dichotomy 517
This question has been attributed by Anick as a conjecture. The answer is
obviously affirmative if k<n(X)®Q is concentrated in odd degrees and a positive
answer is given in [106] for the case ? dim7r2;(-Y) <8> Q = 1.
2i<N
4. // X is a rationally hyperbolic finite simply connected CW complex does Lx
contain a free Lie algebra on two generators?
A positive answer to this question would provide an 'explanation' for the
exponential growth of Lx, and a positive answer was conjectured (separately)
by Avramov and Felix in 1981. The case cato X = 2 was settled in [58] and the
far more general case that depth Lx = 1 was settled positively in [29]. The case
when A' is formal and H*{X) is evenly graded and generated by at most three
elements is settled positively in [13].
5. Suppose X is a simply connected space with rational homology of finite type.
If Heven(X;Q) and the image of the Hurewicz homomorphism are both finite
dimensional does it follow that H*(X;Q) is finite dimensional?
This is trivially true for formal spaces, because in this case a generating space
for the algebra H*(X;Q) is dual to the image of the Hurewicz homomorphism.
If Heven{X; Q) = 0 then Baues' theorem [19] states that X is rationally a wedge
of spheres, which implies a positive answer in this case too.
This question has been referred to in the literature as the Omnibus Conjec-
ture', the origin of the terminology being a false proof produced by the second
author during a particularly slow (omnibus) train ride from Leuven to Louvain
in 1981.
6. Suppose X is a simply connected rationally hyperbolic finite CW complex of
dimension n. Are there numbers ? > 0 and C > 1 such that
s+n-l
? dim7TiPO<8>Q> XCS , all s > 1 ?
i-s+l
A positive answer to this question was conjectured by the authors in the early
1980's. The gap theorem (Theorem 33.3) establishes the weaker (!) inequality
with XCS replaced by 1. An important case where there is a positive answer
is established by Lambrechts in [104]. When X is rationally hyperbolic the
exponential growth of Theorem 33.2 implies that the Hilbert series ?/,(??)(?)
has radius of convergence ? < 1. Lambrechts gives a positive answer to this
problem when the singularities of H„ (??) (?) on the boundary circle of radius ?
are all poles. There are many examples of such spaces, and no examples where
this condition is known to fail.
7. Is the radical R C Lx of a simply connected finite CW complex X of dimension
n, concentrated in degrees < 2(n — 1), and is dimR < n?
518 39 Seventeen Open Problems
When X is elliptic all of L\ is concentrated in degrees < 2(n — 1), and
dimLx < 2dim(jLx)even < catX < n, which motivated the authors to con-
jecture a positive answer to this question in 1982. A weaker question asks:
Are all rational Gottlieb elements of X of degree < 2n — 1 ?
and in the same vein,
If X is rationally hyperbolic is there an a € (Lx)even of degree < n—l
such that ad ? is not locally nilpotent?
The authors believe that the answer to both questions should be yes.
8. Do the rational homotopy types of the configuration spaces of a simply con-
nected compact manifold M depend only on the rational homotopy type of M?
The configuration space of k points in M is the subspace of points (??,..., xk) e
? ? · · · ? ? such that Xi ? Xj for i ? j. In [22] it is shown that the ratio-
nal homology of the configuration space depends only on the rational homotopy
type of M and in [103] and [150] a positive answer is given for smooth complex
projective varieties.
9. Let X be a finite simply connected CW complex. Is X rationally elliptic if and
only if for each prime number ? some pr annihilates all the p-primary torsion
???,(?)?
A positive answer to this question was conjectured by J.C. Moore in the 1970's,
and the Moore conjecture has been a focus of research in unstable homotopy
ever since. In [117] it is shown that rationally elliptic spaces satisfy the Moore
condition for all but finitely many primes. In [10] this is shown for 2-cones. The
full Moore conjecture is known for spheres ([148] and [97]). A survey of progress
as of 1988 is given in [138].
10. Let X be a finite simply connected CW complex. Is it true that either
H*(UX;Z) has ? torsion for all but finitely many primes ? or else that Ht(QX;Z)
has ? torsion for only finitely primes p?
Note that if two finite simply connected CW complexes have the same rational
homotopy type then they have the same homotopy type after inverting only
finitely many primes, so that the question above depends only on the rational
homotopy type of X. Moreover, by using 'integral' Sullivan models it is possible
to compute H* (QX; %·(?)) for all but finitely many primes ? directly from a finite
dimensional commutative rational model for X. The question has a positive
answer for rationally elliptic spaces X since [117] shows that then ?* (??;?)
has p-torsion for only finitely many primes.
The Rational Dichotomy 519
11. If X is a simply connected, rationally hyperbolic, finite CW complex do the
betti numbers bi = dim Hi(QXs ; Q) of the free loop space grow exponentially?
A positive answer to this question was conjectured by Vigue in [153], where
it is proved when ?' is a wedge of spheres or a manifold of LS category 2. A
positive answer is given in [105] for non-trivial connected sums of manifolds. This
conjecture is motivated by the fact that the fibration UX —>· Xs1 —>· X admits
a section and the fact that H*(QX;Q) grows exponentially. However there is a
cautionary example in [153] of a rationally elliptic X such that ?/*(??'; Q) grows
like a cubic while Ht (Xs1 ; Q) grows only like a quadratic.
The original interest in the question arises from the fact that the betti num-
bers of Xs can be used to give a lower bound estimate for the number of
geometrically distinct closed geodesies on X as a function of their length [74].
12. Are all simply connected compact riemannian manifolds M with non-negative
sectional curvature rationally elliptic?
A positive answer to this question has been attributed to Bott as a conjecture,
and in [24] a result is established in this direction. A positive answer in general
would solve the Chern-Hopf conjecture that the Euler-Poincare characteristic is
non-negative and the Gromov conjecture [75] that dimH*(M;Q) < 2n, ? =
dim M. It would also shown that the rationally hyperbolic manifold of [89]
with a metric of positive Ricci curvature did not admit a metric of non-negative
sectional curvature.
In a somewhat different direction Paternain [133] conjectures a positive answer
to the question:
Are all simply connected compact riemannian manifolds with com-
pletely integrable geodesic flows rationally elliptic?
13. Let X be a rationally hyperbolic finite simply connected CW complex. Is it
true for some a in the homotopy Lie algebra of ? autx (X) that ad ? is not locally
nilpotent?
Here auti(X) is the monoid of continuous maps f : X —> X homotopic to
the identity, and the homotopy Lie algebra in question is just ?» (auti (X)) ® Q
with the Samelson product. This question is posed by Salvatore in [136]. Note
that if X is rationally elliptic the homotopy Lie algebra ?, (autx (X)) ®Q is finite
dimensional. Thus this question provides another (conjectural) characterization
of the elliptic-hyperbolic dichotomy.
A second question dealing with the rational homotopy type of ? auti (X) was
raised by Schlessinger in 1976:
Does every simply connected space Y have the rational homotopy type
of some ? auti (X)?
520 39 Seventeen Open Problems
14. Suppose given i : X —> Y = X U/ Dn+l and assume H*(Y;Q) is a finite
dimensional algebra not generated by a single element. If f is not inert does
coker ?, (i) <g> Q grow exponentially?
15. Suppose ?, ? and ? are simply connected rational CW complexes with ho-
mology of finite type. Is it true that XxZ~XxY implies ? ~Y?
This question has a positive answer when ?, ? and ? are formal spaces ([25],
[27])·
16. Let X be a simply connected rationally hyperbolic CW complex with homotopy
Lie algebra Lx. Let ?? = dimX and set
k+??-? k+nx-l
rk= ? dim(Lx)i and mk = ]T dim (LX/[LX, Lx))i .
i-k+1 i=k+l
Does nik/rk —> 0 as k —> oo?
This question is motivated by the theorems that show that Lx has 'lots'
of non-vanishing Lie brackets and by computer experiments that suggest that
[Lx, Lx] is large. A positive answer would assert that in sufficiently large degrees
[Lx,Lx] was arbitrarily close to all of Lx.
A stronger version of the question asks
Is lim (mk/rkI/h =0?
fc-+oo
and this is closely related to a problem in [105]:
If (AV, d) is the Sullivan minimal model for X does the Hilbert series
for AV have a strictly smaller radius of convergence than the Hilbert
series for H(AV, d2) = Ext^* (Q, Q) ?
17. Find an explicit number ? € @,1) that is not the radius of convergence of
the Hilbert series Ht(UX;Q)(z) of the loop space homology of a finite simply
connected CW complex.
There are, up to homotopy type, only countably many simply connected finite
CW complexes. Thus only countably many numbers, ?, appear as the radius
of convergence of H*(UX;Q)(z) in the question above. However the series for
Sn+1 ? Sn+1 V · · · V 5n+1 (r copies) is A - rzn)~l with radius of convergence
(l/rI/". Thus the numbers ? appearing as radii of convergence form a dense
subset of [0,1]. Moreover these numbers ? can be transcendental as is shown by
an example in [8].
References
[1] J.F. Adams, On the cobar construction. Proc. Nat. Acad. Sei USA 42
A956), 409-412.
[2] J.F. Adams and P.J. Hilton, On the chain algebra of a loop space. Com-
ment. Math. Helvetia 30 A956), 305-330.
[3] C. Allday and S. Halperin, Lie group actions on spaces of finite rank Quart
J. Math. Oxford B) 29 A978), 69-76.
[4] C. Allday and V. Puppe, Bounds on the torus rank, in Transformation
groups, Poznam 1985. Lecture Notes in Mathematics 1217, Springer-\;erlag
A986), 1-10.
[5] C. Allday and V. Puppe, Cohomological Methods in Transformation
Groups. Cambridge Studies in Advanced Mathematics 32. Cambridge Uni-
versity Press A993).
[6] D. Anick, A counterexample to a conjecture of Serre. Annais of Math. 115
A982), 1-33, and 116 A982), 661.
[7] D. Anick, Non-commutative graded algebras and their Hubert series. Jour-
nai of Aigebra 78 A982), 120-140.
[8] D. Anick, Diophantine equations, Hubert series and Undecidable spaces.
Annais of Main. 122 A985), 87-112.
[9] D. Anick, A loop space whose homology has torsion of all orders. Pacific
J. Math. 123 A986), 257-262.
[10] D. Anick, Homotopy exponents for spaces of category two. in Algebraic
Topology - Rational Homotopy, Lecture Notes in Math. 1318 A988), 24-
52.
[11] D. Anick, Hopf algebras up to homotopy. J. of Amer. Math. Soc. 2 A989),
417-453.·
[12] M. Aubry, Homotopy Theory and Models. DMV Seminar Band 24,
Birkhauser, 1995.
[13] L. Avramov, Free Lie subalgebras of the cohomology of local rings. Trans.
Amer. math. Soc. 270 A982), 589-608.
[14] L. Avramov, Local Algebra and Rational Homotopy. In iiomotopie
Algebrique ei Algebre Locale. Asterisque 113-114, Societe Mathematique
de France A984), 15-43.
[15] L. Avramov, Torsion in loop space homology. Topology 25 A986), 155-157.
522 REFERENCES
[16] L. Avramov and S. Halperin, Through the looking glass; a dictionary be-
tween rational homotopy theory and local algebra, in Algebra, Algebraic
Topology and their Interactions, Stockholm 1983 Lecture Notes in Mathe-
matics 1183, Springer Verlag 1986, 1-27.
[17] I.K. Babenko and LA. Taimanov, On non formal simply connected sym-
plectic manifolds, Preprint series, math.SG: 9811167v3, 17 Dec. 1998.
[18] J. Barge, Structures differentiates sur les types d'homotopie rationelle
simplement connexes. Ann. Scient. Ec. Norm Sup. 9 A976), 1-33.
[19] J.H. Baues, Rational homotopietypen. Manuscripta Math. 20 A977), 119-
131.
[20] H. Baues, Algebraic Homotopy. Cambridge University Press, 1989.
[21] H. Baues and J.M. Lemaire, Minimal models in homotopy theory. Math.
Ann. 225 A977), 219-242.
[22] M. Bendersky and S. Gitler, The cohomology of certain function spaces.
Trans. Amer. Math. Soc. 326 A991), 423-440.
[23] C Benson and C.S. Gordon, Kahler and symplectic structure on nilmani-
folds. Topology 27 A988), 513-518.
[24] M. Berger and R. Bott, Sur les varietes a courbure strictement positive.
Topology 1 A962), 301-311.
[25] R. Body and R. Douglas, Rational homotopy and unique factorization.
Paci?cJ. of Math. 75 A978), 909-914.
[26] R. Body and R. Douglas, Unique factorization of rational homotopy types.
Paci?c J. of Math. 90 A980), 21-26.
[27] R. Body, M. Mimura, H. Shiga and D. Sullivan, p-universal spaces and
rational homotopy types. Comment. Math. Helv. 73 A998), 427-442.
[28] R. Bogvad and S. Halperin, On a conjecture of Roos. Lecture Notes in
Math 1183 A986), 120-127.
[29] R. Bogvad and C. Jacobsson, Graded Lie algebras of depth one.
Manuscripta Math. 66 A989), 153-159.
[30] A.K. Bousfield and V.K.A.M. Gugenheim, On PL de Rham theory and
rational homotopy type. Memoirs of the Amer. Math. Soc. 179 A976)
[31] E. Brown, Twisted torsion product, I. Annals of Math. 69 A959), 223-242.
[32] E. Cartan, La topologie des groupes de Lie. Actualites Scientifiques et
industrielles, 358. Paris Hermann, 1936.
Rational Homotopy Theory 523
[33] H. Cartan, Notions d'algebre differentielle; applications aux groupes de Lie,
aux varietes ou opere un groupe de Lie. Colloque de Topologie (Espaces
Fibres) Bruxelles A950), Liege et Paris A951), 15-27.
[34] H. Cartan, La transgression dans un groupe de Lie et dans un espace fibre
principal. Colloque de Topologie (Espaces Fibres) Bruxelles A950), Liege
et Paris A951), 57-71.
[35] H. Cartan and S. Eilenberg, Homological Algebra. Princeton Universty
Press, 1956.
[36] H. Chen, Nonexistence of negative weight derivations on graded Artin al-
gebras: A conjecture of Halperin. J. Algebra 216 A999), 1-12.
[37] K.T. Chen, Iterated integrals of differential forms and loop space homology.
Annals of Math. 97 A973), 1033-1035.
[38] C. Chevalley and S. Eilenberg, Cohomology theory of Lie groups and Lie
algebras. Trans. Amer. Math. Soc. 63 A948), 83-124.
[39] L.A. Cordero, M. Fernandez and A. Gray, Symplectic manifolds with no
Kahler structure. Topology 25 A986), 375-380.
[40] O. Cornea, Cone length and LS category. Topology 33 A994), 95-111.
[41] O. Cornea, There is just one cone length. Trans. Amer. Math. Soc. 344
A994), 835-848.
[42] P. Deligne, P. Griffiths, J. Morgan and D. Sullivan, Real homotopy theory
of Kahler manifolds. Invent. Math. 29 A975), 245-274.
[43] G. de Rham, Sur l'analysis situs des varietes a n dimensions. Journal de
Mathematiques Pures et Appliquees 10 A931), 115-200.
[44] J. Dugundji, TopoJogy. Allyn and Bacon, Boston, 1965.
[45] N. Dupont, A couterexample to the Lemaire-Sigrist conjecture, Topology
38 A999), 189-196.
[46] N. Dupont, Problems and Conjectures in rational homotopy theory: A
survey. Expo. Math. 12 A994) 323-352.
[47] W.G. Dwyer, Strong convergence of the Eilenberg-Moore spectral se-
quence. Topology 13 A974), 255-265.
[48] S. Eilenberg and S. MacLane, On the group ?(?,?) I. Annals of Math.
58 A953), 55-106.
[49] S. Eilenberg and J.C. Moore, Homology and fibrations I : cotensor product
and its derived fonctors. Comment. Math. Helvetia 40 A986^ 199-236.
524 REFERENCES
[50] Y. Felix, Caracteristique d'Euler homotopique. Note aux Comptes-Rendus
de l'Academie des Sciences de Paris 291 A980), 303-306.
[51] Y. Felix, La Dichotomie Elliptique-Hyperbolique en Homotopie Ra-
tionnelle. Asterisque 176, Soc. Math. France, 1989.
[52] Y. Felix et S. Halperin, Rational LS category and its applications. Trans.
Amer. Math. Soc. 273 A982), 1-38.
[53] Y. Felix and S. Halperin, Formal spaces with finite dimensional rational
homotopy. Trans. Amer. Math. Soc. 270 A982), 575-588.
[54] Y. Felix, S. Halperin, C. Jacobsson, C. Lofwall and J.-C. Thomas, The
radical of the homotopy Lie algebra. Amer. J. of Math. 110 A988), 301-
322.
[55] Y. Felix, S. Halperin and J.M. Lemaire, The rational L.S. category of
products and of Poincare duality complexes. Topology 37 A998), 749-756.
[56] Y. Felix, S. Halperin, J.-M. Lemaire and J.-C. Thomas, Mod ? loop space
homology. Inventiones Math. 95 A989), 247-262.
[57] Y. Felix, S. Halperin and J.-C. Thomas, L.S. categorie et suite spectrale
de Milnor-Moore (une nuit dans le train). Bull. Soc. Math. France 111
A983), 89-96.
[58] Y. Felix, S. Halperin and J.-C. Thomas, Sur l'homotopie des espaces de
categorie 2. Math. Scand. 55 A984), 216-228.
[59] Y. Felix, S. Halperin and J.-C. Thomas, Loop space homology of spaces
of LS category one and two. Math. AnnaTen 287 A990), 377-386.
[60] Y. Felix and J.-C. Thomas, Sur la structure des espaces de L.S. categorie
deux. Illinois J. Math. 30 A986), 175-190.
[61] J. Friedlander and S. Halperin, An arithmetic characterization of the ra-
tional homotopy groups of certain spaces. Inventiones Math. 53 A979),
117-133.
[62] T. Ganea, Lusternik-Schnirelmann category and strong category. Illinois
J. Math. 11 A967), 417-427.
[63] T. Ganea, Induced fibrations and cofibrations. Trans. Amer. Math. Soc.
127 A967), 442-459.
[64] M. Ginsburg, On the Lusternik-Schnirelmann category. Annab of Math.
77 A963), 538-551.
[65] A. Gomez Tato, Modeles minimaux resolubles. Journal of Pure and Applied
Algebra 85 A993), 43-56.
Rational Homotopy Theory 525
[66] A. Gomez-Tato, S. Halperin and D. Tanre, Non simply connected homo-
topy theory. Preprint .
[67] D. Gottlieb, Evaluation subgroups of homotopy groups. Amer, j ot Math
91 A969), 729-756.
[68] M. Greenberg, Lectures on Algebraic Topology. W.A. Benjamin A966)
[69] W. Greub, S. Halperin and R. Vanstone, Connections, Curvature and Co-
ijomoJogy I. Academic Press A972).
[70] W. Greub, S. Halperin and R. Vanstone, Connections, Curvature and Co-
homoiogy I. Academic Press A973).
[71] W. Greub, S. Halperin and R. Vanstone, Connections, Curvature and Co-
homology M. Academic Press A976).
[72] P.A. Griffiths and J.W. Morgan, Rational Homotopy Theory and Differ-
ential Forms, Progress in Mathematics, 16, Birkhauser, 1981.
[73] D. Gromoll and W. Meyer, Periodic geodesies on a compact riemannian
manifold. J. Differentia] Geometry 3 A969), 493-510.
[74] M. Gromov, Homotopical effects of dilatations. J. Differentia] Geometry
13 A978), 303-310.
[75] M. Gromov, Curvature, Diameter and Betti numbers. Comment. Math.
Helvetia 56 A981), 179-195.
[76] K. Grove and S. Halperin, Contributions of rational homotopy theory to
global problems in geometry. PufaJ. I.H.E.S. 56 A982), 379-385.
[77] K. Grove and S. Halperin, Dupin hypersurfaces, group actions and the
double mapping cylinder. J. Differential Geometry 26 A987), 429-459.
[78] A. Haefliger, Rational homotopy of the space of sections of a nilpotent
bundle. Trans. Amer. Math. Soc. 273 A982), 609-620.
[79] R. Hain, Jnterated integrais and Jiomotopy Periods. Memoirs of the Amer.
Math. Soc. 291 A984).
[80] R. Hain, The geometry of the mixed Hodge structure on the fundamental
group. In Algebraic Geometry, Bowdoin, PSPM, 46 A987), 247-282.
[81] R. Hain, The de Rham homotopy theory of complex algebraic varieties.
K-theory 1 A987), 271-324 and 481-497.
[82] S. Halperin, Lectures on Minimal models. Me'moires de la societe
Mathematiques de France 230 A983).
526 REFERENCES
[83] S. Halperin, Rational homotopy and torus actions. In Aspects of topology,
Mem. H. Dowker, London Math. Soc. Lect. Note Ser. 93 A985), 293-306.
[84] S. Halperin and J.-M. Lemaire, Suites inertes dans les algebres de Lie
graduees. Math. Scand. 61 A987), 39-67.
[85] S. Halperin and J.-M. Lemaire, Notions of category in differential algebra.
Lecture Notes in Mathematics, 1318, A988), 138-154.
[86] S. Halperin and J.D. Stasheff, Obstructions to homotopy equivalences. Adv.
in Math. 32 A979), 233-279.
[87] S. Halperin et M. A^igue-Poirrier, The homology of a free loop space. Pacific
J. of Math. 147 A991), 311-324.
[88] K. Hasegawa, Minimal models of nilmanifolds. Proc. Amer. Math. Soc.
106 A989), 65-71.
[89] M. Hernandez-Andrade, A class of compact manifolds with positive Ricci
curvature. Proc. Symp. Pure Math. 27 A975), 73-87.
[90] K. Hess, A proof of Ganea conjecture for rational spaces. Topology 30
A991), 205-214.
[91] K. Hess, A history of rational homotopy theory. In History of Topology,
Ed. I.M. James, Elsevier Sciences, 1999.
[92] G. Hochschild and J.P. Serre, Cohomology of Lie algebras. Annals of Math.
57 A953), 591-603.
[93] W. Hurewicz, Beitrage zur Topologie der Deformationen I-I W. Proc.
Koninkl. Akad. Wetenschap Amsterdam. 38 A935), 111-119, 521-528,
and 39 A936), 117-126, 215-224.
[94] K. Iwasawa, On some types of topological groups. Ann. of Math. 50 A949),
507-748.
[95] N. Iwase, Ganea's conjecture on Lusternik-Schnirelmann category. Bull.
London Math. Soc. 30 A998), 623-634.
[96] I. James, On category in the sense of Lusternik-Schnirelmann. Topology 17
A978), 331-348.
[97] I. James, On the suspension triad of a sphere. Ann. of Math. 63 A956),
407-429.
[98] B. Jessup, Rational L.-S. category and a conjecture of Ganea. Journal of
Pure and Applied Algebra 65 A990), 57-67.
[99] B. Jessup, Holonomy nilpotent fibrations and rational Lusternik-
Schnirelmann category. Topology 34 A995), 759-770.
Rational Homotopy Theory 527
[100] ?. Jessup and A. Murillo-Mas, Approximating rational spaces with elliptic
complexes and conjecture of Anick. Pacific J. Math. 181 A997), 269-280.
[101] R. Kaenders, On De Rhasn Homotopy Theory for Plane Algebraic Curves
and their Singularities. Thesis, Nijmegen, 1997.
[102] J. L. Koszul, Sur un type d'algebre differentielle en rapport avec la trans-
gression. Colloque de Topologie (Espaces Fibres) Bruxelles 1950, Liege et
Paris A951), 73-81.
[103] I. Kriz, On the rational homotopy type of configuration spaces. Annals of
Mathematics 139 A994), 227-237.
[104] P. Lambrechts. Analytic properties of Poincare series of spaces. Topology
37 A998), 1363-1370.
[105] P. Lambrechts, Croissance des nombres de Betti des espaces de lacets,
These Louvain-La-Neuve A995)
[106] L. Lechuga and A. Murillo, Complexity in rational homotopy. Topology
39 A999), 89-94.
[107] D. Lehmann, Theorie homotopique des formes differentielles. Asterisque
45, Soc. Math. France, 1977.
[108] J.-M. Lemaire, A ?nite complex whose rational homotopy is not finitely
generated. Lecture Notes in Mathematics 196 A971), 114-120.
[109] J.-M. Lemaire, Algebres connexes et homologie des espaces de lacets. Lec-
ture Notes in Math. 422, Springer-Verlag, 1974.
[110] J.-M. Lemaire, "Autopsie d'une meurtre" dans l'homologie d'une algebre
de chaines. Ann. Scient. Ec. Norm. Sup. 11 A978), 93-100.
[Ill] J.M. Lemaire and F. Sigrist, Sur les invariants d'homotopie rationnelle lies
a la L.S. categorie. Comment. Math. Helvetia 56 A981), 103-122.
[112] C. Lofwall and J.E. Roos. Cohomologie des algebres de Lie graduees et
series de Poincare-Betti non rationelles. Note aux Comptes-Rendus Acad.
Sei. Paris 29?'A98?), 733-736.
[113] G. Lupton, Note on a conjecture of Stephen Halperin's. Springer Lecture
Notes in Math. 1440 A990), 148-163.
[114] G. Lupton and J.F. Oprea, Symplectic manifolds and formality. Journal
Pure and Applied Algebra. 91 A994) 193-207.
[115] G. Lupton and J. Oprea, Cohomologically symplectic spaces: toral actions
and the Gottlieb group. Trans. Anm. Math. Soc. 347 A005), 961-288.
528 REFERENCES
[116] L. Lusternik and L. Schnirelmann, Methodes topologiques dans les
problemes variationeis. Actualites scientifiques et industrielles, 188, Paris,
Hermann, 1934.
[117] C. McGibbon and C. Wilkerson, Loop space of finite complexes at large
primes. Proc. Amer. Math. Soc. 96 A986), 698-702.
[118] S. MacLane, Homology, Springer-Verlag, Berlin and New York, 1975.
[119] M. Majewski, A proof of the Baues-Lemaire conjecture in rational homo-
topy theory. Rend. Circ. Mat. Palermo. Suppl. 30 A993), 113-123.
[120] M. Markl, Towards one conjecture on collapsing of Serre spectral sequence,
in Geometry and physics, Proc. 9th Winter Sch., Srni/Czech. 1989, Suppl.
Rend. Circ. Mat. Palermo, 22 A990), 151-159.
[121] C.R.F. Maunder, Algebraic Topology. Van Nostrand Reinhold Company,
London,1970.
[122] J.P. May, Simplicial object in Algebraic Topology. University of Chicago
Press, 1967.
[123] W. Meier, Rational universal fibrations and flag manifolds. Math. Ann.
258 A982), 329-340.
[124] S.A. Merkulov, Formality of canonical symplectic complexes and Frobenius
manifold. Preprint series, math. SG:9805072, 15 May 1998.
[125] J. Milnor, Construction of the universal bundles, I and I. Annals of Math.
63 A956), 272-284 and 430-436.
[126] J. Milnor, The geometric realization of a semisimplicial complex. Annals
of Math. 65, A957) 357-352.
[127] J. Milnor and J.C. Moore, On the struture of Hopf algebras. Annals of
Math. 81 A965), 211-264.
[128] J. Milnor and J.D. Stasheff, Characteristic classes. Princeton Univ. Press,
1974.
[129] J. Neisendorfer and P. Selick, Some examples of spaces with or without
exponents, in Current trends in Algebraic Topology, CMS Conf. Proc. 2
A982), 343-357.
[130] K. Nomizu, On the cohomology of compact homogeneous spaces of nilpo-
tent Lie groups. Annals of Math. 59 A954), 531-538.
[131] J. Oprea, Gottlieb Groups, Group Actions, Fixed Points and Rational
Homotopy. Lecture Notes series 29, Research Institute of Math. Seoul Na-
tional University A995).
Rational Homotopy Theory 52g
[132] S. Papadima et L. Paunescu, Reduced weighted complete intersection and
derivations. Journal of Algebra 183 A996), 595-604.
[133] G. Paternain, On the topology of manifolds with completely integrable
geodesic flows. Ergodic Theory Dyn. Syst. 12 A992), 109-122.
[134] D. Quillen, Homotopical algebra. Lecture Notes in Math. 43, Springer-
Verlag, 1967.
[135] D. Quillen, Rational homotopy theory. Annals of Math. 90 A969), 205-
295.
[136] P. Salvatore, Rational homotopy nilpotency of self-equivalences. Topoiogy
Appl, 77 A997), 37-50.
[137] H. Scheerer and D. Tanre, Fibrations a la Ganea. Bull. Belg. Math. Soc. 4
A997), 333-353.
[138] P. Selick, Moore conjectures. In Algebraic Topology - Rational Homotopy,
Lecture Notes in Math. 1318 A988), 219-227.
[139] J.P. Serre, Lie algebras and Lie Groups. New-York, Bejamin, 1965.
[140] J.P. Serre, Algebre Locale - Multipliates. Lecture Notes in Math. 11,
Springer-Verlag, 1965.
[141] H. Shiga and M. Tezuka, Rational fibrations, Homogeneous spaces with
positive Euler characteristics and Jacobians. Ann. Inst. Fourier 37 A987),
81-106.
[142] E.H. Spanier, Algebraic Topology. McGraw Hill, Academic Press, 1966.
[143] D. Sullivan, Geometric Topology- 1 : Localization, Periodicity, and Galois
Symmetry. MIT Press, 1970.
[144] D. Sullivan, Infinitesimal computations in topology. Publ. I.H.E.S. 47
A977) 269-331.
[145] D. Tanre, Homotopie Rationnelle : Modeles de Chen, Quillen, Sullivan.
Lecture Notes in Mathematics 1025, Springer-Verlag, 1983.
[146] J.C. Thomas, Rational homotopy of Serre fibrations. Ann. Inst. Fourier
331 A981), 71-90.
[147] J.C. Thomas, Homologie de l'espace des lacets, problemes et questions.
Journal of Pure and Applied Algebra 91 A994), 355-379.
[148] H. Toda, On the double suspension E2. J. Inst. Polytech. Osaka City Univ.
A7 A956), 103-145.
530 REFERENCES
[149] G. Toomer, Lusternik-Schnirelmann category and the Milnor-Moore spec-
tral sequence. Math. Zeitschrift 138 A974), 123-143.
[150] ?. Totaro, Configuration spaces of algebraic varieties. Topology 35 A996),
1057-1067.
[151] G. Triantafillou, Equivariant Rational Homotopy Theory. In Equivariant
homotopy and cohomology theory, C.B.R.M. 91 A996), 27-32.
[152] M. Vigue-Poirrier et D. Sullivan, The homology theory of the closed
geodesic problem. Journal of Differential Geometry 11 A976), 633-644.
[153] M. Vigue-Poirrier, Homotopie rationnelle et nombre de geodesiques
fermees. Ann. Scient. Ecole Norm. Sup. 17 A984), 413-431.
[154] J.M. Wahl, Derivations, automorphisms and deformations of quasi-
homogeneous singularities. In Proc. Symp. Pure Math. 40 A983), 613-624.
[155] C. Watkiss, Cohomology of principal bundles in semisimplicial theory. The-
sis, Toronto A974).
[156] A. Weil, Sur les theoremes de de Rham. Comment. Math. Helv. 26 A952),
119-145.
[157] G. Whitehead, Elements of homotopy theory. Graduate Text in Math. 61
Sprinberg-Verlag, New York Inc., 1978.
[158] G. Whitehead, The homology suspension. Colloque de Topologie
Algebrique, Lou\'ain A957), 89-95.
[159] J.C.H. Whitehead, On adding relations to homotopy groups. Ann. of Math.
42 A941), 409-428.
[160] J.C.H. Whitehead, Combinatorial homotopy I and I. Bull. Amer. Math.
Soc 55 A949), 213-245 and 453-496.
[161] O. Zariski and P. Samuel, Commutative Algebra. Graduate Texts in Math.
28, Springer-Verlag, 1975.
Index
Alexander-Whitney map 53, 477
Bar construction 268, 277
of an enveloping algebra 305
Borel construction 36
Minimal Sullivan model for Bq
217
Sullivan model for the Borel con-
struction 219
Bundle
Associated fibre bundle 36
Fibre bundle 32
Milnor's universal G-bundle 36
Principal G-bundle 35
CW complex 4
CW ^-complex 102
Cellular approximation theorem
9
Cellular chain model 60
Cellular chain model for a fibra-
tion 90
Cellular chain model of a space
59
Cellular map 5
Cellular models theorem 12
Product of 7
Quotient of 7
Relative CW complex 5
Subcomplex 5
Wedge of CW complexes 7
Whitehead lifting lemma 12
Cartan-Eilenberg-Che\ralley construc-
tion on a dgl
(see chain coalgebra on a dgl)
301
Cartan-Serre Theorem 231
Cell attachment 173
Sullivan model 173
Cellular approximation theorem 9
Cellular chain model 59, 60
Chain algebra 46
Chain algebra on a loop space
343
Chain coalgebra
Chain coalgebra on a dgl 301
Chain coalgebra on a dgl, C. (L)
301
Chain complex 43
C,(L;UL) 302,305
Cellular chain complex 59
Chain complex of .4-modules 273
Chain complex of t/X-modules
464
Chain complex on a dgl 305
Normalized singular chain com-
plex 52
of finite length 475
Cobar construction 271
Cochain algebra 46
C*{X;k) 65
Cochain algebra APL(X) 121
Cochain algebra on a dgl 313,
314
Cochain complex 43
Normalized singular cochain com-
plex 65
Cofibration 15
Cohomology
De Rham Theorem 128
Lie algebra cohomology 320
Relative singular cohomology 66
Singular cohomology 65
Commutathre model 116
Complex projective space 6
Lie model 333
Cone length 360, 362
Rational cone length 370, 372,
374,382,388
Cup product 65
Cup-length 366
Rational cup length 370, 387
De Rham Theorem 128
Depth of a Lie algebra 492, 493
Depth of an algebra 474, 486
Differential graded algebra 46
532
INDEX
dga Homotopy 344
Direct product 47
Fibre product 47
Tensor product 47
Differential graded coalgebra 48
Differential graded Hopf algebra 297
Differential graded Lie algebra 296
Module 296
Differential model on a dga
Semifree model 183
Differential module on a dga 46
Equivalence 68
Homotopy of maps 68
Lifting property 70
Semifree module 69, 262
Dupont example 388
Eilenberg-MacLane space 62
Simplicial construction 246
Sullivan model 201
Euler-Poincare characteristic 49, 444
Existence of models 182
Exponents 441
Ext 275, 465
Fibration 23
G-(Serre) fibration 28
Lifting property 23, 24
Path space fibration 29
Serre fibration 23, 33
Fibre of the model 196
Formal dimension 441
Ganea spaces 357, 366
Ganea fibres 357, 479
Ganea's fibre-cofibre construction 355
Ginsburg's Theorem 399
Global dimension 474
Gottlieb groups 377, 378, 392
Grade 474
Projective grade 474, 476, 481
Graded algebra 43
Commutative 45
Free commutative 45
Tensor algebra 45
Tensor product 44
Graded coalgebra 47, 299
Coderivation 301
Primitively cogenerated 299
Graded Hopf algebra 288
Primitwe element 288
Graded Lie algebra 283
Abelian Lie algebra 283, 285
Adjoint representation 284, 422
Derivation 284
Derived sub Lie algebra 283
Free graded Lie algebra 289, 316
Lie algebra cohomology 320
Lie bracket 283
Module 283
Morphism 283
Product 284
Representation 284
Universal em'eloping algebra 285
Graded module 40
Suspension 41
Tensor product 41
Graphs (ra-colourable) 440
Hess' Theorem 393
Hubert series 49
Holonomy fibration 30, 342, 378
Holonomy representation
for a fibration 415, 418
for a relative Sullh'an algebra
416, 418
Homogeneous space 218, 435
Sullivan model 219
Homology
Singular homology 52
Homotopy
Based homotopy 3
Homotopy rel 3
Homotopy equivalence 3, 242
Weak homotopy equivalence 12,
14, 58
Homotopy fibre 30, 196, 334
Homotopy groups 10, 208, 452
Cartan-Serre Theorem 231
Long exact sequence 26, 213
Rational homotopy groups 453
Homotopy Lie algebra
of a space 292, 294, 295, 325,
432
Rational Homotopy Theory
533
of a Sullivan algebra 295, 317,
475
of a suspension 326
Hurewicz homomorphism 58, 210, 231,
234, 293, 326
Iwase example 407
Jessup's Theorem 424
Kahler manifold 162
Koszul-Poincare series 444
Lie group 216
Matrix Lie group 219
Right invariant forms on a Lie
group 161
Sullivan model for a Lie group
161
Sullivan model for the orbit space
X/G 219
Lie model for a space 322
Cellular Lie model 323, 331
Free Lie model 322, 324, 383
Lie model for a wedge 331
Lie model of a wedge of spheres
331
Lie model of the product 332
Lie models for adjunction spaces
328
Lie model of a dgl 309
Free Lie extension 310
Minimal Lie model 311
Minimal Lie model for a Sulli-
van algebra 316
Localization 108
TMocal group 102
"P-local space 102
CW-p-complex 102
Cellular localization 108
Relative CW-p complex 104
Loop space 29, 340
??-spaces 476
Chain algebra on a loop space
88, 343, 347
Decomposability 449
LS category 380
Rational product decomposition
228, 229
Loop space homology
Basis 230
Primitive subspace 230
Loop space homology algebra
Milnor Moore Theorem 293
Loop space homology Ht{UX;k)
Depth 486
Loop space homology algebra H* (QX
224, 232, 458
Lusternik-Schnirelmann category (of
algebras and modules)
of a differential module 401
of a Sullivan algebra 381, 384,
488
Lusternik-Schnirelmann category (of
spaces) 351, 362, 406, 486
of a continuous map 352, 481
of free loop spaces 376
of loop spaces 380
of Postnikov fibres 376
Rational LS category 386, 387,
388
Rational LS category of a space
370, 371, 374, 432
Rational LS category of smooth
manifolds 396
Whitehead LS category 353
Mapping Theorem 375, 389, 411
Milnor-Moore Theorem 293
Nilmanifolds 162
Poincare-Birkoff-Witt Theorem 286,
287
Polynomial differential forms 122
Product length, nil 381, 382
Projective dimension 278, 474, 481
Quasi-isomorphisms 152
Quillen construction C(C,d) 306, 307
Quillen plus construction 212
Radius of com'ergence 459
Rational elliptic space 434
Rational hyperbolic space 452
Rational space 102
Rational cellular model 111
Rational homotopy equivalence
mOQQ
534
INDEX
Rational homotopy type 111
Rationalization 108, 386
Regular sequence 437
Resolution
i/,(fiy)-free resolution of Ht(F)
483
Eilenberg-Moore resolution 279,
480
Projective (free) resolution 274
Semifree resolution 69, 71, 278
The Milnor resolution of Ic 478.
480
Simplex
Degeneracy map 52
Degenerate 52
Face map 52
Linear simplex 52
Singular n-simplex 52
Simplicial morphism 117
Simplicial object 116
Extendable simplicial object 118,
240
Milnor realization 238
Simplicial cochain algebra 117
Simplicial set 117
Spatial realization 248, 386
Homotopy groups 250
of a relath'e Sullh'an model 248
Spectral sequence 260, 261
Comparison Theorem 265
Eilenberg-Moore spectral sequence
280
Filtered module 261, 266
Hochschild-Serre spectral sequence
464
Milnor-Moore spectral sequence
317, 318, 319, 399
Odd spectral sequence 438
Sphere 2, 7, 11
:P-local n-sphere 102
Fundamental class 58
Homology algebra H.(Q.Sn;k)
225, 234
Homology Lie algebra of a wedge
of spheres 326
Lie model of a sphere 322
Lie model of a wedge of spheres
331
Loop space homology of a fat
wedge 462
Loop space homology of a wedge
of spheres 460
Model of the loop space Q.Sk
200
Rational homotopy groups of spheres
210
Sullivan model for a sphere 142
Whitehead product 178
Spherical fibration 202
Sullivan algebra 138
Pure Sullh'an algebra 435
Relative Sullivan algebra 181, 262
Sullh'an model for a commutath'e cochain
algebra 138, 141
Formality 156
Homotopy of maps 139, 148.150,
153
Lifting lemma 148, 184, 186
Linear part of a morphism 152,
295
Linear part of the differential
141
Minimal Sullivan model 154,186
Sullivan model for a map
Fibre 181, 192
Model of a pullback fibration 204
Model of fibrations 195
Model of spherical fibrations 202
Model of the path space fibra-
tion 226
Sullivan model for a space 138, 141
Contractible Sullivan model 139
Minimal Sullivan model 138, 146,
' 154
Model for a cell attachment 173
Model for a product 142
Model for a suspension 171
Model for a wedge 155
Model for an if-space 143
Models for smooth manifolds 213
Rational Homotopy Theory
535
Quadratic part of the differen-
tial 175, 176
The homotopy groups 171, 208
Sullivan realization functor 247
Sullivan representative for a map 154
Symmetric spaces 162
Symplectic manifolds 163
Tensor algebra 268
Tensor coalgebra 268
The set of homotopy classes 151
Toomer's invariant 366, 367
of a Sullivan algebra 381, 386,
391, 400
of a differential module 401
Rational Toomer invariant 370,
374, 386, 387, 432
Topological group 35
Classifying space 36
Orbit space for a group action
35
Topological monoid 28
Action of 28
Chain algebra on 88
Topological space
Ji-space 143
fc-space 1
ra-cone 359
Adjunction space 3, 8, 328
Cell attachment 4
Cofibre 352
Conformai space 334, 388
Compact-open topology 1
CW complex 4
Filtered space 5
Formal space 156, 162, 163, 388
Homotopy fibre 30, 78
Join 21
Mapping cylinders 8
Mapping spaces 1
Moore path space 29
NDR-pair 15, 20
Product of 1, 7
Quotient of 1, 7
Reduced cone 352
Retract 3,15
Smash product 21
Suspension 3, 7, 20, 359
Wedge of spaces 2, 7, 462
Well-based space 15
The construction A(K) 118
The simplicial cochain algebra
124
Tor 275, 465
Differential Tor 281
Torus action 435
Uniqueness of minimal models 152
Universal enveloping algebra 285,486
of a differential graded Lie alge-
bra 296
Poincare-Birkoff-Witt Theorem
286, 287
Weak homotopy type 12, 14
Whitehead lifting lemma 12
Whitehead product 175, 176,
293
Whitehead-Serre Theorem 94
Wordlength 140
in a tensor algebra 290
in Sullivan model 158, 262, 318
232,