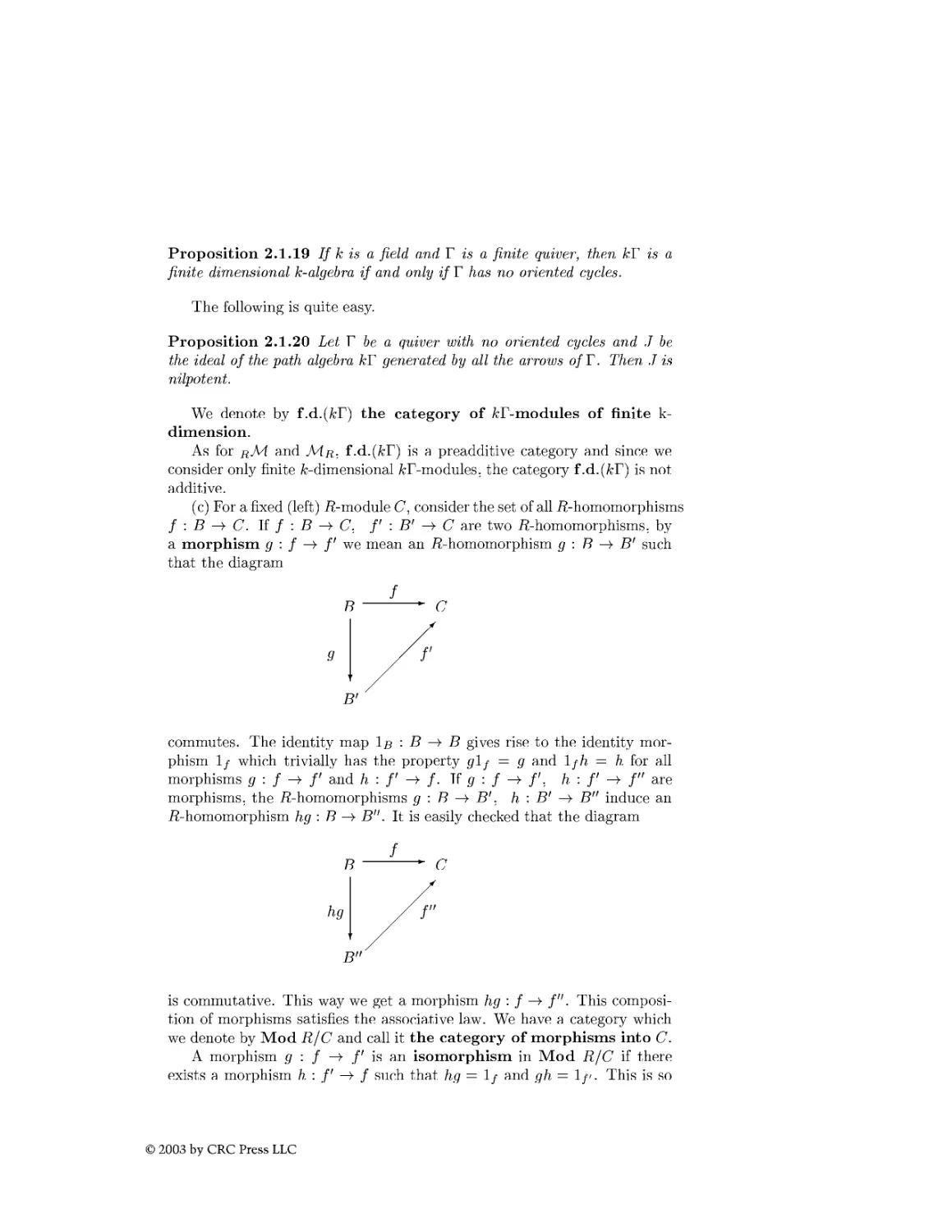
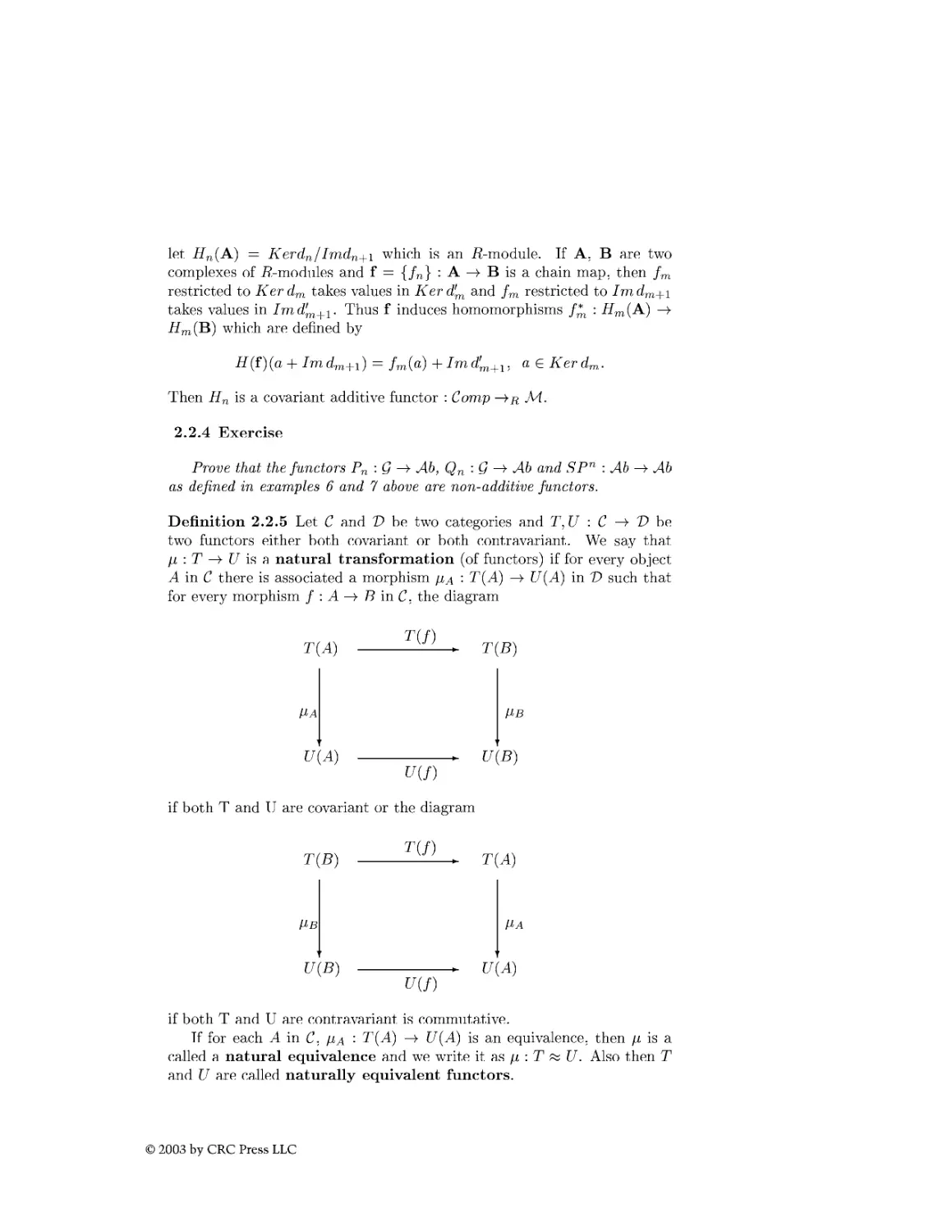
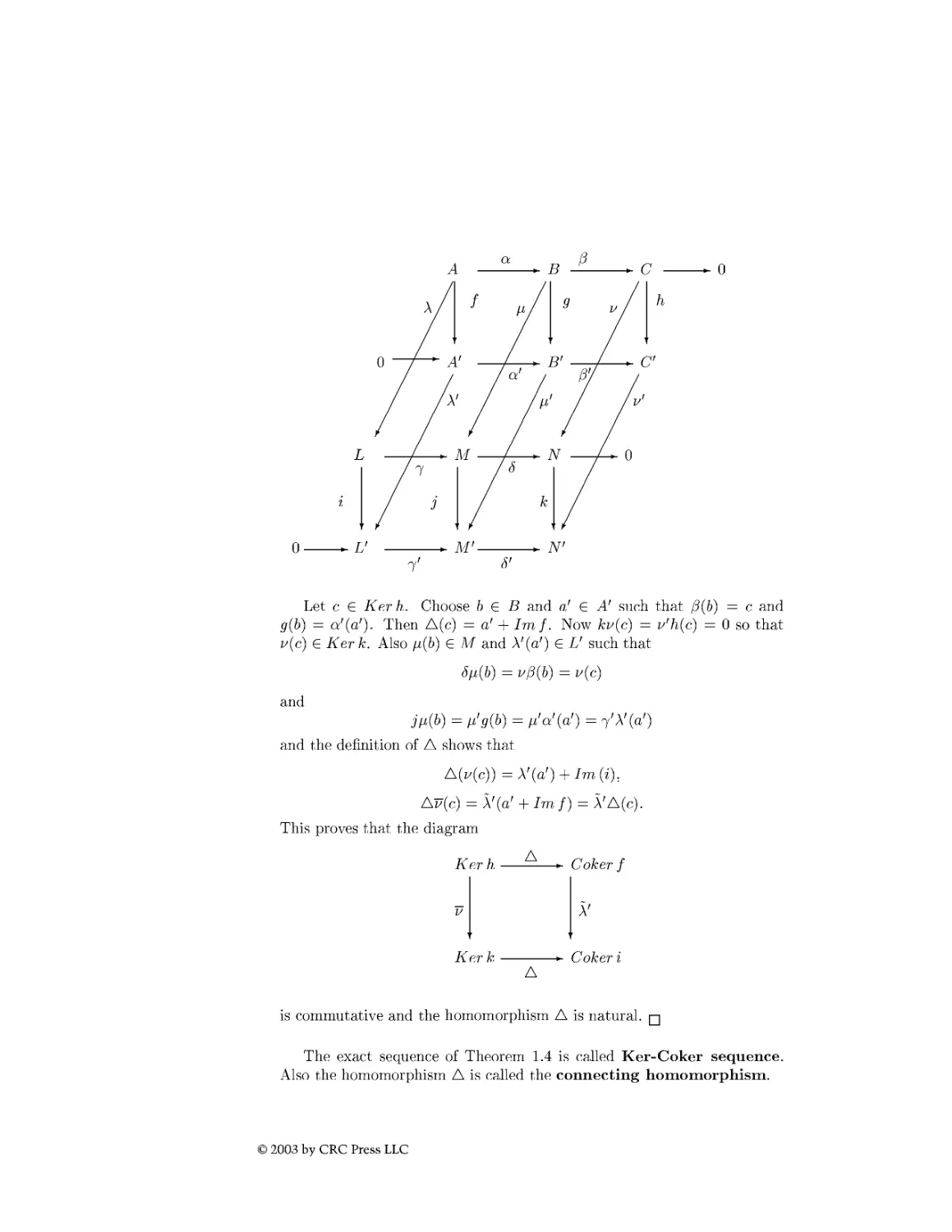
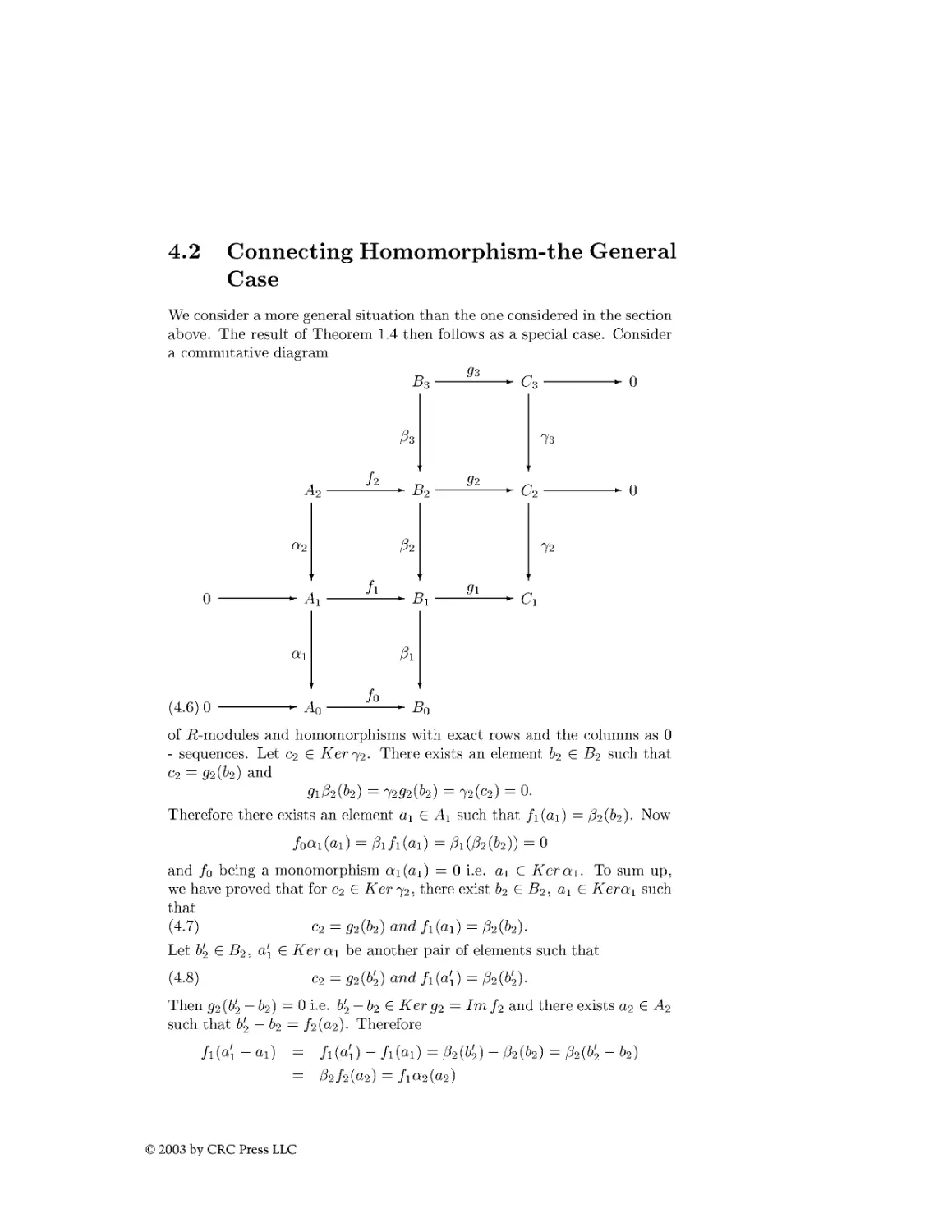
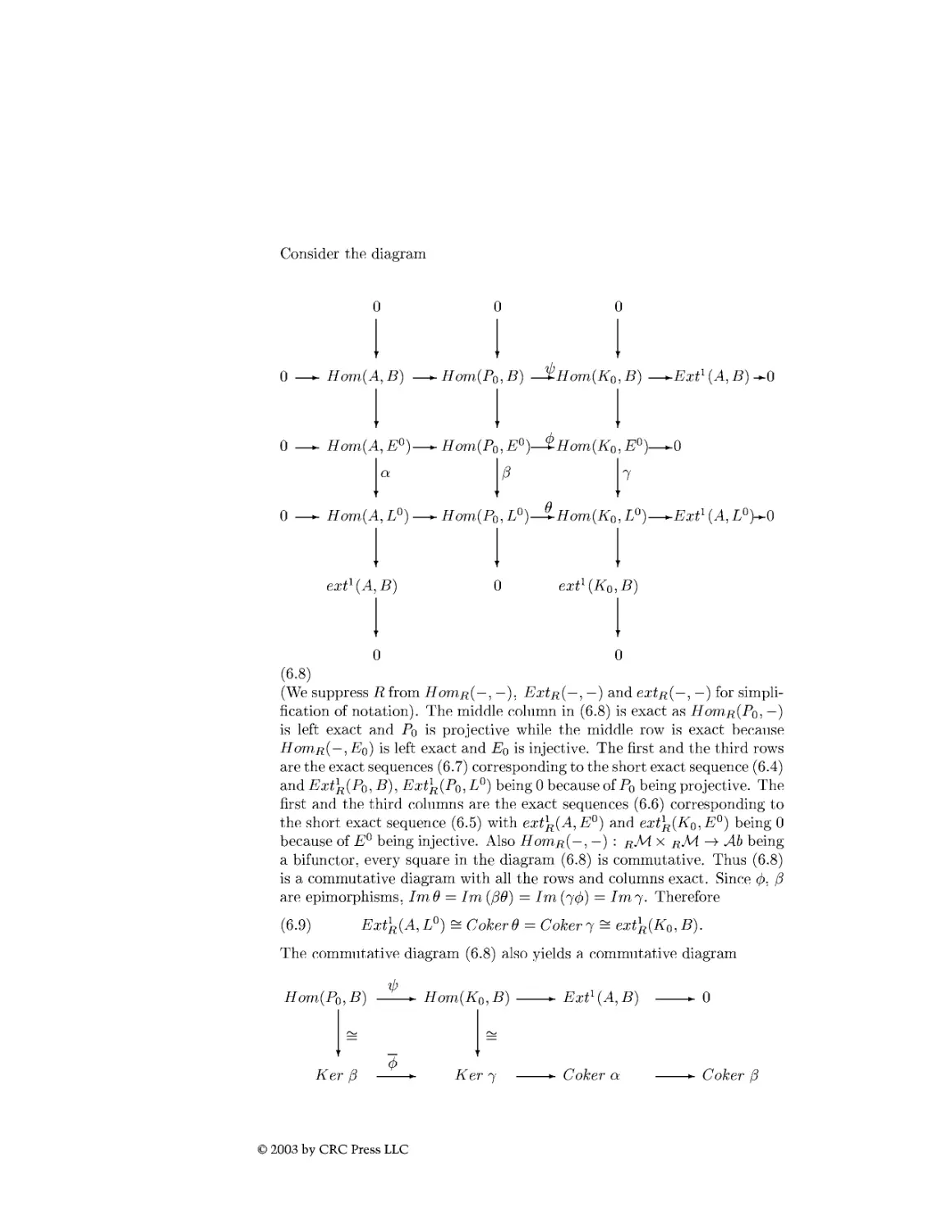
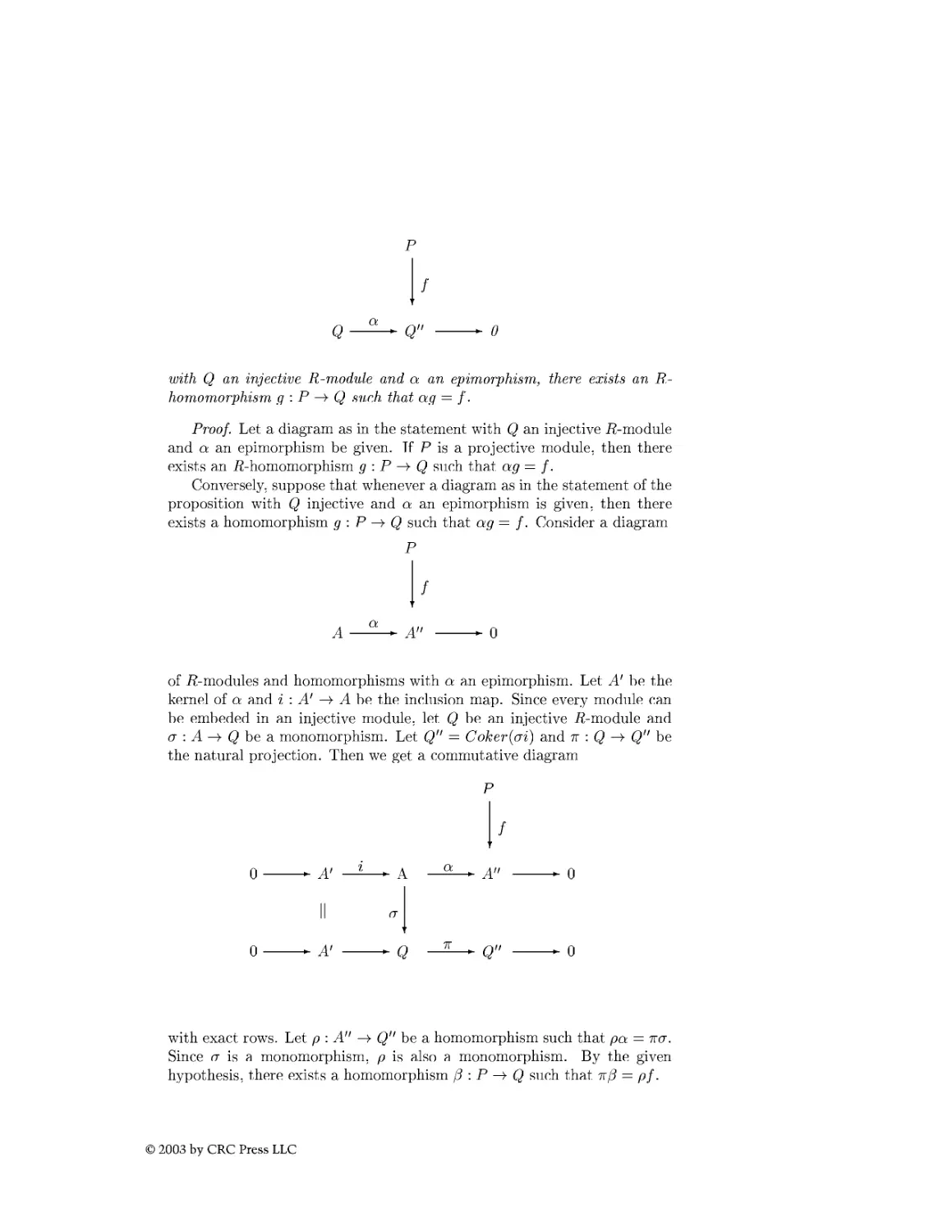
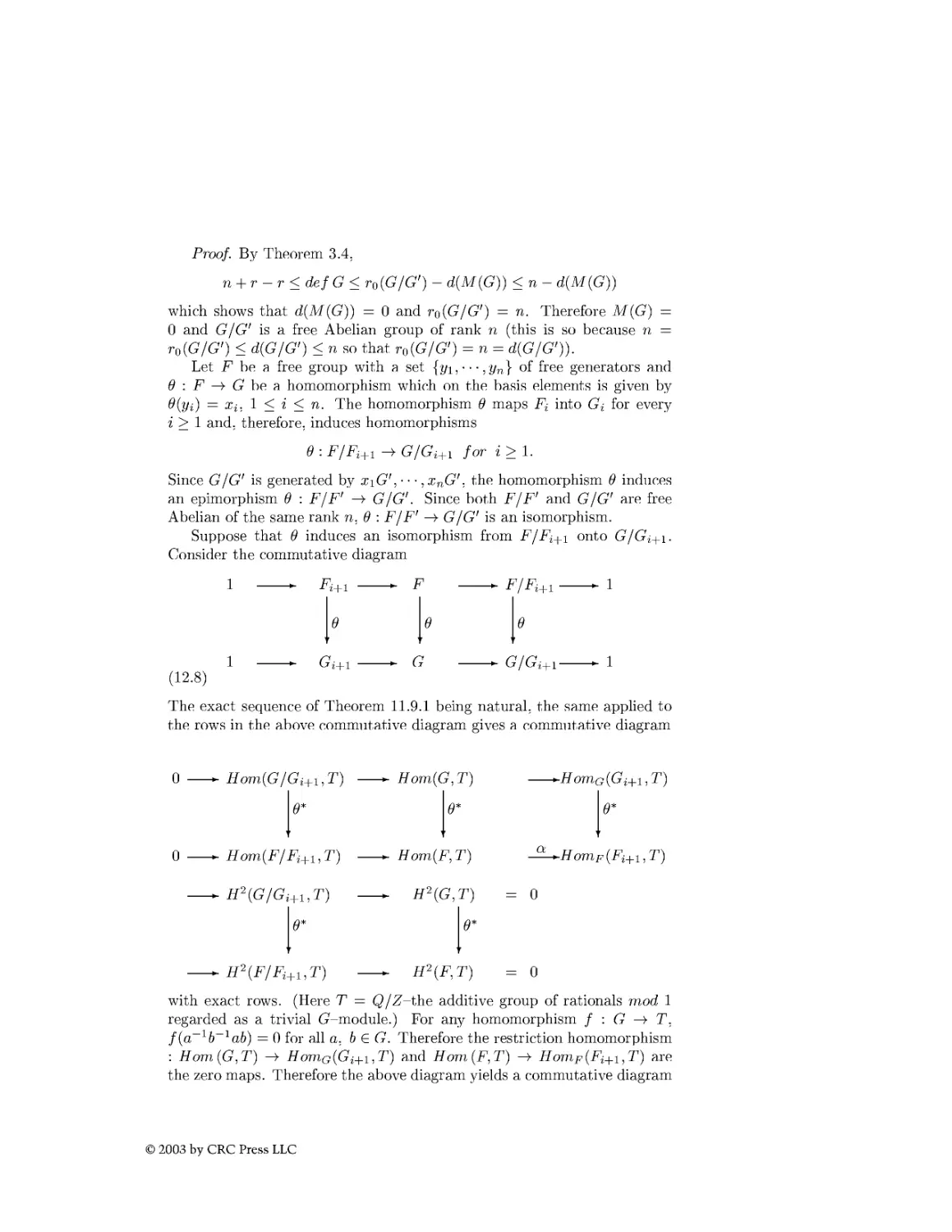
Текст
K
CHAPMAN & HALL/CRC
Monographs and Surveys in
Pure and Applied Mathematics I 30
AN ELEMENTARY
APPROACH TO
HOMOLOGICAL ALGEBRA
L R. VERM AN I
CHAPMAN & HALL/CRC
A CRC Press Company
Boca Raton London New York Washington, D.C.
© 2003 by CRC Press LLC
CHAPMAN & HALL/CRC
Monographs and Surveys in Pure and Applied Mathematics
Main Editors
H. Brezis, Universite de Paris
R.G. Douglas, Texas A&M University
A. Jeffrey, University of Newcastle upon Tyne (Founding Editor)
Editorial Board
R. Aris, University of Minnesota
G.I. Barenblatt, University of California at Berkeley
H. Begehr, Freie Universitdt Berlin
P. Bullen, University of British Columbia
R.J. Elliott, University of Alberta
R.P. Gilbert, University of Delaware
R. Glowinski, University of Houston
D. Jerison, Massachusetts Institute of Technology
K. Kirchgassner, Universitdt Stuttgart
B. Lawson, State University of New York
B. Moodie, University of Alberta
L.E. Payne, Cornell University
D.B. Pearson, University of Hull
G.F. Roach, University of Strathclyde
I. Stakgold, University of Delaware
W.A. Strauss, Brown University
J. van der Hoek, University of Adelaide
© 2003 by CRC Press LLC
Library of Congress Cataloging-in-Publication Data
Vermani, L. R. (Lekh R.)
An elementary approach to homological algebra / Lekh R. Vermani.
p. cm. — (Chapman & Hall/CRC monographs and surveys in pure and applied math)
Includes bibliographical references and index.
ISBN 1-58488-400-2 (alk. paper)
1. Algebra, Homological. I. Title. II. Chapman & Hall/CRC monographs and surveys in
pure and applied mathematics.
QA169.V48 2003
512'.55—dc21 2003046075
This book contains information obtained from authentic and highly regarded sources. Reprinted material
is quoted with permission, and sources are indicated. A wide variety of references are listed. Reasonable
efforts have been made to publish reliable data and information, but the author and the publisher cannot
assume responsibility for the validity of all materials or for the consequences of their use.
Neither this book nor any part may be reproduced or transmitted in any form or by any means, electronic
or mechanical, including photocopying, microfilming, and recording, or by any information storage or
retrieval system, without prior permission in writing from the publisher.
The consent of CRC Press LLC does not extend to copying for general distribution, for promotion, for
creating new works, or for resale. Specific permission must be obtained in writing from CRC Press LLC
for such copying.
Direct all inquiries to CRC Press LLC, 2000 N.W. Corporate Blvd., Boca Raton, Florida 33431.
Trademark Notice: Product or corporate names may be trademarks or registered trademarks, and are
used only for identification and explanation, without intent to infringe.
Visit the CRC Press Web site at www.crcpress.com
© 2003 by CRC Press LLC
No claim to original U.S. Government works
International Standard Book Number 1-58488-400-2
Library of Congress Card Number 2003046075
Printed in the United States of America 1234567890
Printed on acid-free paper
© 2003 by CRC Press LLC
To My Grand Son Siddharth
© 2003 by CRC Press LLC
Preface
Homological algebra arose from many sources in Algebra and Topol-
Topology. However, the subject appeared as a full-fledged subject in its own
right in 1956 when the first book on the subject and still a masterpiece
by H. Cartan and S. Eilenberg appeared. More books have appeared on
the subject since then, notably by D.G.Northcott. S. MacLane. P.J.Hilton
and U. Stammbach. ,T.,T. Rotman. C. A. Wiebel. However, none of these
could be adopted as a textbook for a student coming across the subject for
the first time. The author felt this difficulty while teaching a one semester
course on the subject at Kurukshetra University during the last, few years.
The students found it. hard in the absence of a suitable text book. The
present text is a result of the lectures given at Kurukshetra during which
time books by Northcott. Rotman and Hilton and Stammbach were freely
used while lecturing. The material covered in the book may be adopted
for a two semester course, while a one semester course could be based on
the first seven chapters. The book shall also be useful for researchers who
like to use the subject in their study. Complete detailed proofs are given
to make the book easy for self study.
The book aims at giving just a basic course on the subject and is by
no means exhaustive. Several important areas in the subject have not. even
been touched upon.
We now briefly describe the contents of the book. The book starts
with a brief account of modules, homomorphisms of modules and elemen-
elementary properties of tensor products of modules. Direct and inverse limits of
families of modules and pull back and push out diagrams are also intro-
introduced. The concept of categories and functors is introduced in Chapter 2.
Although homomorphisms and tensor products of modules are studied in
Chapter 1. functorial properties of Horn and Tensor Product are discussed
here.
Homological algebra may be aptly described as a study of derived func-
functors of (additive) functors- in particular the functors Horn and Tensor prod-
product. Derived functors of additive functors are defined in chapter 5. For
defining these the existence of projective and injective resolutions for every
module is needed and the same is also established in this chapter. Chapters
3 and 4 are preparatory for defining and studying derived functors. Derived
functors of tensor product are called torsion functors (Tor^) while those
of Horn are called extension functors (Ext1^). Some special properties of
the functors (Tor^) and (Ext7^) are studied in chapters 6 and 7. Torsion
and extension functors can also be defined for categories not having enough
projectives or enough injectives (contrary to the category of modules) and
also derived functors of non-additive functors can be defined but we do not
discuss these here.
Chapter 8 gives a connection between the ring of scalars and the mod-
r things, it is proved that (i) over hereditary
rings distinction between projective and injective modules disappears while
(ii) over Dedekind domains the same happens for divisible and injective
modules. For studying the (co) homology of direct sum of two groups, it is
necessary to know a connection between the homologies of two complexes
X. Y and that of the complex X®Y. This relationship is given by the Kun-
neth formula a special case of which as needed later is considered in Chapter
9. Chapter 10 studies projective and injective dimensions of modules and
left and right global dimensions of rings. Only simple characterizations of
these are given. However, the equality of left and right global dimensions of
a ring which is both right and left Noetherian is proved. A characterization
of left global dimension of a left Artin ring is also given.
A study of a special case of torsion and extension functors i.e. the case
when the ring of scalars is the integral group ring ZG of a group G is taken
up in chapter 11. These special cases are Hn(G,A) and Hn(G,A) the nth
homology group of G and the nth cohomology group of G with coefficients
in a G-module A. Homology and cohomology groups can also be defined
through (co)cycles and (co)boundaries. That, the two approaches lead to
the same objects, up to isomorphism, is established by introducing the Bar
resolution. The last three sections of the chapter are developed to mainly
obtain information about the second cohomology group. The connection
between the study of H2 (G, A) and the study of group extensions of A by G
is discussed. The 5-term exact sequence of Hochschild and Serre connecting
the cohomology of a group G to those of a normal subgroup H of G and the
quotient group G/H and some extensions of this sequence are obtained.
The last chapter, as applications of homological methods, gives two
purely group theoretic problems. One of these is a result, of Gasclmtz that,
every non-Abelian finite p-group has an outer automorphism of p-power
order and the other result, as shown by Magnus is that, a group having a
free presentation with n + r generators and r relations which can also be
generated by n elements is a free group of rank n.
I would like to express my sincere thanks to my teachers (i) I.B.S.Passi
who introduced me to the subject and (ii) D.Rees. I am thankful to my
research student. Manoj Kumar for his help in transferring the manuscript
from M.S.Word to LaTex. Without his help, it would, perhaps, not have
been possible to give the manuscript the present shape. T like to place on
record my appreciation for (i) my colleagues Vivek Sharma and Pradeep
Kumar for their help and (ii) the authorities of JMIT. Radaur for providing
facilities during the last, stage of the project.
© 2003 by CRC Press LLC
Contents
Bibliography
1 MODULES
1.1
1.2
1.3
1.4
1.5
1.6
1.7
Modules
Free Modules
Exact Sequences
Homomorphisms
Tensor Product, of Modules
Direct and Inverse Limits
Pull Back and Push Out
2 CATEGORIES AND FUNCTORS
2.1 Categories
2.2 Functors
2.3 The Functors Hom and Tensor
3 Projective and Injective Modules
3.1 Projective Modules
3.2 Injective Modules
3.3 Baer's Criterion
3.4 An Embedding Theorem
4 Homology of Complexes
4.1 Ker-Coker Sequence
4.2 Connecting Homomorphism-the General Case
4.3 Homotopy
5 Derived Functors
5.1 Projective Resolutions
5.2 Injective Resolutions
© 2003 I
6 Torsion and Extension Functors
6.1 Derived Functors-Revisited
6.2 Torsion and Extension Functors
6.3 Some Further Properties of Tor^
6.4 Tor and Direct Limits
7 The Functor Ext%
7.1 Ext1 and Extensions
7.2 Baer Sum of Extensions
7.3 Some Further Properties of Ext\
8 Hereditary and Semihereditary Rings
8.1 Hereditary Rings and Dedekind Domains
8.2 Invertible Ideals and Dedekind Rings
8.3 Semihereditary and Prufer Rings
9 Universal Coefficient Theorem
9.1 Universal Coefficient Theorem for Homology
9.2 Universal Coefficient Theorem for Cohomology
9.3 The Kenneth Formula, - a Special Case
10 Dimensions of Modules and Rings
10.1 Projectively and Injectively Equivalent Modules
10.2 Dimensions of Modules and Rings
10.3 Global Dimension of Rings
10.4 Global Dimension of Noetherian Rings
10.5 Global Dimension of Artin Rings
11 Cohomology of Groups
11.1 Homology and Cohomology Groups
11.2 Some Examples
11.3 The Groups H*(G,A) and H0(G,A)
11.4 The Groups H^G^A) and Hi(G,A)
11.5 Homology and Cohomology of Direct Sums
11.6 The Bar Resolution
11.7 Second Cohomology Group and Extensions
11.8 Some Homomorphisms
11.9 Some Exact Sequences
12 Some Applications
12.1 An Exact Sequence
12.2 Outer Automorphisms of p-groups
12.3 A Theorem of Magnus
© 2003 by CRC Press LLC
Chapter 1
MODULES
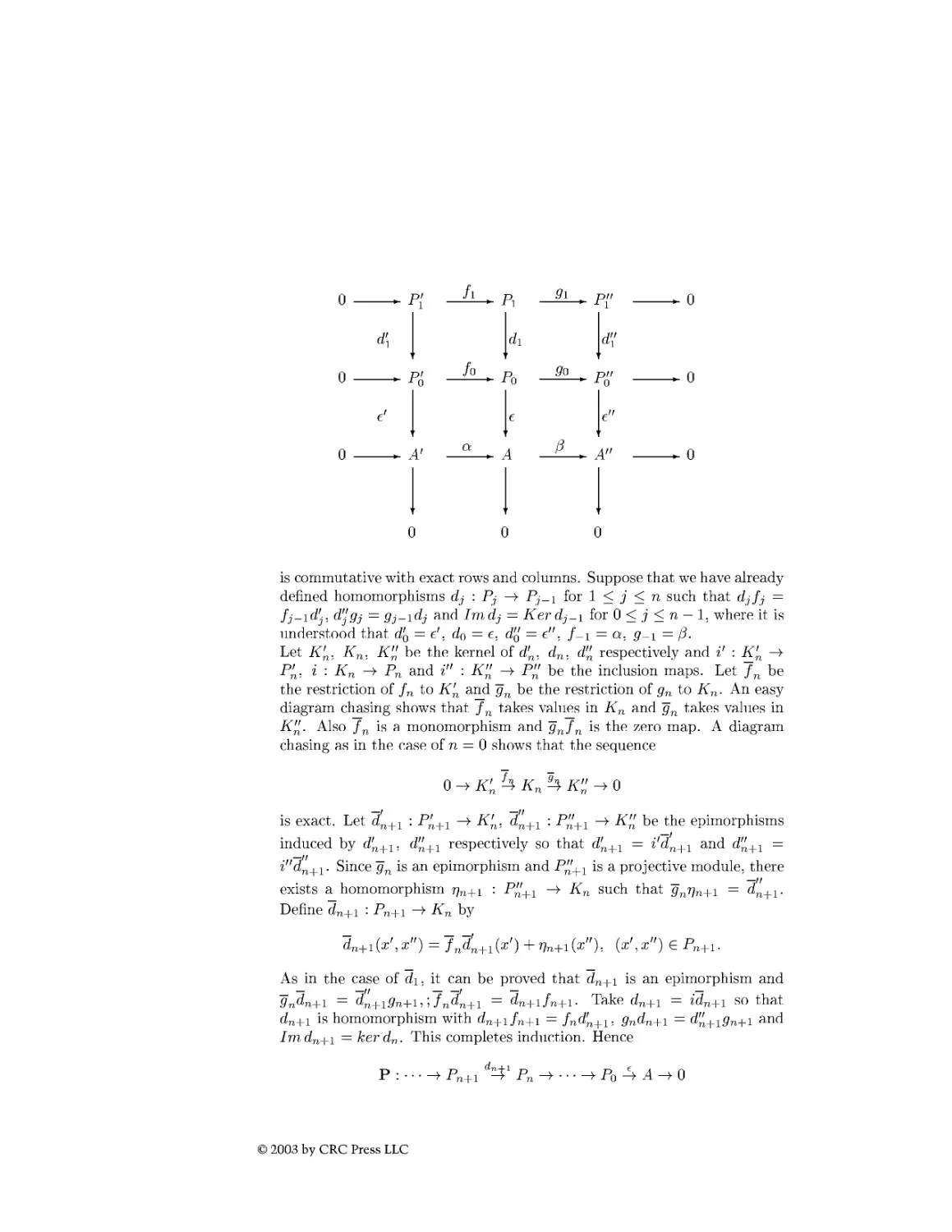
This chapter is preparatory in nature and we give some results on modules.
We define a free module and prove that every (left) i?-module is homomor-
phic image of a free i?-module. When A. B are left i?-modules and C is a
right i?-module. the Abelian groups Homn(A,B) and C ®r B are defined
and some properties of these are obtained. The concepts of direct, limit,
inverse limit, pull back and push out are introduced and some properties
of these are obtained.
1.1 Modules
Definition 1.1.1 Let R be a ring with identity. An additive Abelian group
M is called a left i?-module if there exists for every element r ? R. and
every element a ? M, a uniquely determined element ra of M such that
the following hold :
(i) (r + s)a = ra + sa for every r,s ? R,a ? M:
(ii) (rs)a = r(sa) for every r,s ? R,a ? M:
(iii) r(a + b) = ra + rb for every r ? R,a,b ? M:
(iv) la = a for every a ? M. where 1 denotes the identity of the ring R.
1.1.2 Similarly, an additive Abelian group M is called a right i?-module
if for every r ? R and a ? M there exists a unique element, ar of M such
that, the following hold :
(«)' a(r + s) = ar + as for every r,s ? R,a ? M:
(ii)' (ar)s = a(rs) for every r,s ? R,a ? M:
(iii)' (a + b)r = ar + br for every r ? R,a,b ? M:
(iv)' al = a for every a ? M. where 1 denotes the identity of the ring R.
1.1.3 If R is a commutative ring with identity and M is a left R-module,
let us define a.r for a ? M, r ? R by a.r = ra. Then, for a ? M. r,s ? R.
a.(rs) = (rs)a = (sr)a = s(ra) = s(a.r) = (a.r).s
© 2003 by CRC Press LLC
Properties («)'. (Hi)' and (iv)' of a right R-module can be checked even
more easily. Thus M has been converted into a right i?-module. On the
other hand, a right i?-module can similarly be made into a left i?-module.
In view of this when the ring R is commutative we talk of only an R-module
rather than a right i?-module or left i?-module.
(i) Let S be a ring and R. be a subring of S. Tf r G R, s G 5. then
r,s G S and. therefore, rs G S. Using the associative law for multiplication
in S and the distributive laws we find that S becomes a left i?-module. We
can also similarly regard 5 as a right i?-module. In particular, we find that
every ring R can be regarded as a right as well as a left i?-module.
(ii) Let A be an Abelian group written additively. For an integer n and
a ? A define na to be 0 if n = 0. a + a + ¦ ¦ ¦ + a(n times) if n is positive
and na = (—n)(—a) if n is negative. A then becomes a Z-module.
(iii) Let R be a ring and G be a group written multiplicatively. Let RG
denote the set of all finite formal sums J2geG rgg, rg G R and rg = 0 except
for finite number of elements g G G. For J2geG rgg, J2geG sgg G RG, say
that Yl eG rg9 = E eG sg9 ^ an<^ on^y ^ rg = sg f°r eveiT 9 ? G. For
: rg9, J2QeG sg9 e RG, define
and
With these compositions RG becomes a ring. Identifying r G R with
the element rl of RG. where 1 denotes the identity of the group G. the ring
R. becomes a subring of RG. The ring RG is called the group ring of the
group G over the ring R. By (i) RG becomes a left i?-module as well as a
right i?-module.
(iv) Let R be a ring. G be a group and H be a subgroup of G. The
group ring RH is a subring of the group ring RG and. so. RG is left (as
well as a right) i?iJ-module.
Definition 1.1.4 If M is a left i?-module. a subgroup TV of the additive
group M is called a submodule of M if for every a ? N. r ? R. the
element ra G AT.
Observe that a non-empty subset TV of a left i?-module M is a submodule
of M if and only if (i) for every a,b G N. the element, a — b G TV; and (ii)
ra G N for every r G R,a G N.
1.1.5 Let M be a left iJ-module and /V be a submodule of M. Then /V
being a subgroup of the additive Abelian group M. we have the Abelian
group M/N = {a + N\a G M). the quotient group of M modulo the
subgroup TV. For a? M,r ? R. define
© 2003 by CRC Press LLC
A.1) r(a + N)=ra + N
If a+ N = b + N, then b - a + c for some c G N and r(b + N) = rb + N =
r(a + c) + N = ra + rc + N = ra + N = r(a + N), as re G N. Thus the scalar
product as in A.1) is well defined and M/N becomes a left i?-module and
is called the quotient module of M modulo the submodule N.
1.1.6 Let M, N be two left i?-modules. A map / : Af -»¦ AT is called an
i?-homomorphism or module homomorphism if
f(a +b) = /(a) + f(b) for all a, b G M: and
f(ra) = rf(a) for all r G R, a G M.
An i?-homomorphism / : M —»¦ N is called a monomorphism if the map
/ is one-one, it is called an epimorphism if the map / is onto and it
is called an isomorphism if the map / is both one-one and onto. Two
left i?-modules M and N are said to be isomorphic if there exists an
i?-isomorphism from M to N or N to M.
Let M. N be left i?-modules and / : M —> N be an i?-homomorphism.
Then kernel and image of / are respectively defined by
Kerf = {a G M\f(a) = 0};
Imf = {x G N\x = f (a) for some a G M}
= {f(a)\aGM}.
It is fairly easy to see that. Ker f is a submodule of M and Ira f is a sub-
module of AT. Then cokernel and coimage of/ are respectively defined by
Coker f = N/Im f and Coim f = M/Kerf.
1.1.7 Exercises
1. If / : M —> N is an i?-homomorphism of left i?-modules. prove that
Ker f is a submodule of M and Ira f is a submodule of N.
2. Prove that, an i?-homomorphism / : M —> N is a monomorphism if
and only if Ker / = 0.
3. If M is a left i?-module and N is a submodule of M. prove that any
submodule of M/N is of the form K/N where K is a submodule of M with
4. If / : M —> N is an i?-homomorphism of left i?-modules. then
M/Ker f = Ira f.
5. If A. B are submodules of a left i?-module M. prove that A n B is a
submodule of M.
6. For submodules A, B of a left i?-module M, define A+B = {a+b\a G
A, b G i?}. Prove that. A + i? is a submodule of M containing both A and
© 2003 by CRC Press LLC
7. For submodules A, B of a left 72-module M, prove that (A + B)/B =
A/A r\B.
8. Tf 7" is a left ideal of R, show that R/I = {r + I\r G R} is a left
7?-module.
9. If S is another ring with identity, an Abelian group M is called an
(R, S')-bimodule if M is a left 7?-module. a right S'-module and for every
r G R, s G 5, a G M, (ra)s = r(as).
The ring R is an (R, 7?)-bimodule.
All R-modules considered shall be left R-modules unless mentioned explicitly
to the contrary.
1.2 Free Modules
1.2.1 Let {Mi}i?l be a family of left 72-modules. Let UieIMi denote the
set of all sequences {xi)i^i. xi G Mi. Tf {xi)i^i, {yi)iei G IJ.je/Mj. say that
(a;,) = (j/j) if and only if xt = yt for every i G T. For (xi)iei. (yi)iei G
nje/Mj. and r G R, define
fe) + (j/i) = (xi + Vi) and r(xi) = (rxi).
With these compositions IL,e/Mj becomes a left i?-module. Observe that
the additive identity of IJ.je/Mj is the sequence (xj)jg/ where Xj = 0 for
every i G 7. We denote this element, by @) or simply 0. Also, for any
(xi) G IIje/Mj. its additive inverse is the element (j/j). where j/j = — Xi for
every i ? T . We write the additive inverse of {xi) as (—xiji^i.
The left R-module n^/Mj is called the direct product of the family
i of left i?-modules.
Let © 5Zie/ -^i denote the subset, of IL,e/Mj consisting of those se-
sequences (xj)jg/ in which Xj = 0 except for a finite number of ?' G 7 .
It is easy to see that. © 5Zie/ -^i ^s a submodule of the 7?-module IIje/Mj.
The left 7?-module © 5Zie/ -^i 1S called the external direct sum of the
family {Mi\iei of left R-modules.
On the other hand, given a left 7?-module M and a family of submodules
\Mi}i?i of M. M is called the (internal) direct sum of the family of
submodules if every a G M can be uniquely written as Xw=i aij; where
ciij G Mij. 1 < i < n. Also each Mi is then called a direct summand of
M.
1.2.2 Exercises
1. Let {Mi}ieI be a family of left R-modules and let M = © Y^iei Mi-
Prove that, for every i G 7. there exists a submodule M[ of M such that
(a) M\ = Mi for every i G 7;
(b) M is the (internal) direct, sum of the family {M/} of submodules of M.
© 2003 by CRC Press LLC
2. Prove that a left i?-module M is direct sum of its submodules Mj. 1 <
i < n, if and only if M = ?"=1 Mi and Mi n E"=i, ^ Mj = M for every
i, 1 < i < n.
1.2.3 Let M be a left B-module and X be a subset of M. If every element
of M can be written as a finite sum ^ riXi,n G R,xt G X then M is said to
be generated by X or that. X generates the left i?-module M. The module
M is said to be finitely generated if X is a finite subset of M. Tf the
subset X consists of a single element x (say), then M is called a cyclic
module generated by x.
Again consider a family {Mi}i&i of i?-modules. For any j G I, define
a.j : Mj -»¦ UieiMi and ttj : UieiMi -»¦ Mj by
"j^j) = (Vk)-. where yk = 0 ior k j^ j and yj = Xj G My,
¦Kj((xi)) = Xj, where Xj G Mj for z G T.
The maps o.j,ttj are i?-homomorphisms and
^{
identity if k = j
0 iik^j
Also every otj is a monomorphism while every ttj is an epimorphism. It is
clear that, every atj takes values in © 5Zie/ -^i so ^^at we have monomor-
phisms a.j : Mj —> © 5Zie/ -^«- Restriction of ttj to the submodule © 5Zie/ -^
of IIje/Mj again yields epimorphisms ttj : © 5Zie/ -^i ~^ Mj.
Let a; G (B^Mi. Suppose that, in x = (xi) the non-zero components
are x^ ,Xi2 ¦ ¦ ¦,Xik. Then TTi(x) = 0 for i $ {i\, ¦ ¦ ¦, ik} and TTi(x) = Xi for
i G {i\, ¦ ¦ ¦, ik}. Therefore J^ aiTTi(x) = x. Hence J^ ajTTj = identity map
of ©EM,.
We next consider the universal property of direct sum and direct prod-
product. We consider the family {Mi}i&i of left i?-modules. © Y^iei Mi the di-
direct sum and H^jMi the direct product of this family with monomorphism
aj : Mj -»¦ UieiMi for every j G / and epimorphism ttj : UieiMi -»¦ Mj for
every j.
—>¦
Theorem 1.2.4 Given any left R-module M and monorphisms fj : Mj
M for every j ? I, then there exists a unique R-homomorphism f :
© Y^iei Mt ^ M such that f otj = fj for every j G /.
Proof. Let x = (xi) G © Eie/ Mi. Since x^ ^ 0 for only finitely many
values of i, we can define / : © X^ej Mj —> M by
The map / is an i?-homomorphism. Consider Xj G Mj for a j G 7.
Then o.j(xj) = (t/j). where j/j = 0 for i 7^ j and j/j = Xj. Therefore
© 2003 by CRC Press LLC
(fuj)(xj) = f{a.j{xj)) = f{yi) = Y^iei fiiVi) = fj(xj)-
Hence / a, = fj for every j ? T.
Let g : ®^Mi —> M be another i?-homomorphism such that g a.j = fj
for every j G T. An x G © E Mi can be written as x = E aj{xj)-, where j
runs over a finite subset, of /. Therefore
showing that g = f. \j
Let A be another left i?-module with monomorphisms /3j : Mj —>¦
A for every j G /. Suppose that, given any left i?-module M and R-
monomorphism gj : Mj —> M for every j ? I. there exists a unique ho-
homomorphism g : A -»¦ Af such that g/3j = g, for every j G 7". Taking
Af = © XI-^j an<^ 5? = ai- we nn<^ that there exists a unique homomorh-
pism a : A -»¦ © ^ Mj such that
A.2) a/Jj = aj /or every j.
On the other hand taking M = A and fj = [ij in Theorem 2.4. there
exists a unique i?-homomorphism [j : © ^ Mj ->¦ A such that
A.3) (iotj = Cj for every j.
A.2) and A.3) together imply
(/3a)/3j = Pj and (aC)aij = ctj for every j G T.
Since IaPj = Pj and lffiy^M Q-j = a.j for every jGl.it follows by unique-
uniqueness of the homomorphism which exists as in Theorem 2.4 and of the ho-
homomorphism which exists as in the case of property of A. we get
Pa = \a and aP = 1 y* M..
Therefore a : A -»¦ © E Mi is an isomorphism with f3 : (B^Mi ^ A &s
its inverse. This proves that, direct, sum of modules is determined uniquely
upto isomorphism by the universal property as in Theorem 2.4.
Theorem 1.2.5 Given any left R-module M and R-epimorphisms gj :
M —> Mj, for every j G /, then there exists a unique homomorphism
g : M —> IIje/Mj such that njg = gj for every j G I.
© 2003 by CRC Press LLC
Proof. Define a map g : M ->¦ II;e/M; by g(x) = (gi(x)),x G M. Then
g is an i?-homomorphism and Hjg = gj.
Let / : M —> n^/Mj be another i?-homomorphism such that tt7/ = gj
for every j G T. For x G M. let /(x) = (xi). Then (?j(a;) = TTjf(x) =
TTj(xi) = Xj for every j ? J. Therefore f(x) = (xi) = (gi(x)) = g(x) and
we have g = f. n
We next prove that direct product of left i?-modules is determined
uniquely upto isomorphism by the universal property as mentioned in The-
Theorem 2.5.
Let A be a left R-module with epimorphisms Xj : A -»¦ Mj. Suppose
that for every left i?-module M and epimorphisms fj : M -»¦ Mj there
exists a unique i?-homomorphism / : M —> A such that Xjf = fj for every
j. By taking M = IL,e/Mj and fj = ttj for every j in the above property
of A, let / : IIje/Mj —> A be the unique i?-homomorphism such that
A.4) Xjf = ttj for every j G T.
On the other hand, taking M = A and gj = Xj in Theorem 2.5. let g : A —>
be the unique i?-homomorphism such that.
A.5) iijg = Xj for every j G I.
Now A.4) and A.5) imply that, gf : UieIMi -> UieIMi and fg : A -> A
are homomorphisms such that.
A.6) TTj(g f) = ttj and Xj(f g) = Xj for every j ? T.
Also 1a '¦ A -»¦ A and lnM; : IIMj -»¦ IIMj are homomorphisms such that
Ajlyt = Aj and TTjlnM; = ttj for every j ? T.
By the uniqueness of the homomorphism in the universal property of IIje/Mj
and of the homomorphism as in the property of A, we get g f = lriM; ,f 9 =
1a which show that. / : n^/Mj —> A is an isomorphism with g : A —>
Mi as its inverse.
Definition 1.2.6 A left R-module F is called a free left R-module on a
basis X ^ /p. if there is a map a : X —> F such that given any map / : X —>
A. where A is any left i?-module. there exists a unique i?-homomorphism
g : F —> A such that. / = go..
The unique homomorphism g : F —> A is said to extend the map / :
X-> A.
We observe that the map a : X —> F is necessarily one-one. Tf not.
suppose that xi,x2 ? X such that a(xi) = a(x2)- Take A = Rx R =
{(r, s)\r, s G R} converted into a left i?-module by defining
(ri,si) + (r2,s2) = (n +r2,si + s2), rur2,Si,s2 G R
© 2003 by CRC Press LLC
and r(ri,s\) = (rri,rs2): r,ri,s\ ? R.
Take / : X -»¦ A the map such that
f{xi) = A,0), f{x2) = @,1) and f(x) = 0 for every x ? X,x ^ Xi,x ^ x2.
Let g : F —> A be the unique i?-homomorphism such that, ga = f. Now
A,0) = f(Xl) = ga(Xl) = ga(x2) = f(x2) = @,1)
which is a contradiction.
Theorem 1.2.7 For every non-empty set X, there exists a free left R-
module F with X as a basis.
Proof. Consider the family \Rx}xex of left i?-modules where Rx = R
for every x ? X. Let F = S)^xeX Rx- Tn view of Exercise 2.2 A).
we can regard F as the internal direct sum of the family of submodules
{Rx}xgx- For x ? X,RX being equal to R. we denote an element r ? R.
when considered as an element, of Rx by rx. Under this assumption, every
element, of F can be uniquely written as a finite sum Y^i=\ rixi-. where
7-j ? R,Xi ? X. Let a : X -»¦ F be the map a(x) = 1.x. Let A be any left
i?-module and / : X -»¦ A be any map . Define g : F -»¦ A by
Clearly g is a well defined i?-homomorphism and f = ga. That g is unique
with f = ga is clear. Hence F is a free left i?-module with X as a basis, q
Observe that, the argument in the proof of the above theorem leads to
an alternative definition of a free module. In view of the map a : X —> F
in the definition of a free module being one-one . we may regard X as
a subset of F. Let A = S)^xeXRx; where Rx = R. for every x ? X.
Let g : F —> A be the unique extension of the inclusion map X —> A to
an i?-homomorphism. Since X may also be regarded as a subset, of A,
g maps Ylrixi onto Ylirixi- Also A having been proved to be free. let.
h : A —> F be the unique i?-homomorphism which extends the inclusion
map X -»¦ F. Now hg : F -»¦ F is a homomorphism which extends the
inclusion map X —> F and identity map F —> F also extends this map.
Therefore hg = 1 which implies that, g is a monomorphism and hence, an
isomorphism. Thus F is a free left i?-module with basis X if and only
if F = ®Y,xexRx-<Rx = R f°r every x ? X. Moreover. F is free with
basis X if and only if every element of F can be uniquely expressed as
Y,nxi,ri ? R.
Theorem 1.2.8 Every left R-module is homomorphic image of a free left
R-module.
© 2003 by CRC Press LLC
Proof. Let M be a left i?-module. Let X be a set the elements of which
are in one to one correspondence with the elements of M. Let the element
of X corresponding to the element a ? M be denoted by xa. Let F be the
free left i?-module with X as a basis. Let / : X —> M be the map given by
f(xa) = a. Let g : F —> M be the unique homomorphism which satisfies
a = f(xa) = g(xa). The homomorphism g is clearly an epimorphism. q
Theorem 1.2.9 Let F be a free left R-module with basis X, a : A —»¦ B
an epimorphism of left R-modules and f : F —»¦ B an R-homomorphism.
Then there exists an R-homomorphism g : F —»¦ A such that ag = f.
Proof. For every x ? X. choose an element. ax ? A such that. f(x) =
a(ax). Define a map /? : X ->¦ A by j3(x) = ax,x ? X. Let g : F ->¦ A be
the unique i?-homomorphism which extends the map /3. Let X ? F. Then
A = Y^irixi-. where n ? R,xt ? X. Therefore
ag{\) = a.
which proves that ag = f. q
1.2.10 Examples
1. Observe that R is always a free left i?-module with a basis consisting
of a single element. It is also a free right i?-module with a basis consisting
of a single element.
2. Every submodule of a free left i?-module need not be free. Consider
R = Z/6Z-the ring of integers modulo 6. 2Z/6Z = {0 + 6Z, 2 + 6Z, 4 + 6Z}
is a submodule of the free i?-module R. The ring R. is of order 6 while
the order of the module 2Z/6Z is 3. Therefore the module 2Z/6Z cannot
be direct, sum of any copies of R. Hence 2Z/QZ is not a free R = Z/6Z-
module. However, every submodule of an i?-module when R is a principal
ideal domain is free (we shall come back to it. later).
3. Let R. be a commutative ring and R[X] be the polynomial ring in
the variable X over R. Then R[X] is a free i?-module with basis {X'}i>0.
Proposition 1.2.11 Let G be a group, H a subgroup of G and X be a
right transversal (i.e. set of right r.oset representatives) of H in G. Then
RG is a free left RH-module.
Proof. Every element g of G can be uniquely written as hx, h ? H,x ?
X. Therefore any element Yl ri9i ? R(* can be written as Y2 ri9i =
Y2i i"ihiXi and nhi ? RH. This shows that. RG is generated as an RH-
module by X.
© 2003 by CRC Press LLC
Suppose that Y^i=iaixi = 0; where a, ? RH. Let a, = Y^JLi rijhij-
Then we have 0 = X^Ej rijhij)xi.
Consider the elements {%?«}«,.? of G occuring in the above linear com-
combination. Since hij 7^ hik for j 7^ k. therefore hijXi 7^ hikXi for j ^ k. Also
Xj,a;j for 7 7^ / being in distinct, right cosets of H in G, h^Xi 7^ h^xi for
7 7^ /. Therefore all the elements in the set {h^Xi} are distinct. There-
Therefore, Y^i jrijhijXi = 0 shows that rjj = 0 for all i,j which implies that
Ylj rijhij = 0 f°r aH J or a^ = 0 for all i. This proves that every element of
RG can be uniquely written as ^ aiXi, aj G RH. Hence RG is a free left
i?iJ-module. q
1.2.12 Exercise
Prove that the additive group Q of rational numbers is not a free Z-
module.
1.3 Exact Sequences
Consider a sequence
A.7) A^bAC
where A,B,C are i?-modules and / : A -»¦ B. g : B ->¦ C are R-
homomorphisms. We say that, sequence A.7) is a 0-sequence if Im f C
Ker g while it. is said to be exact if Im f = Ker g.
A sequence
A-8) ¦¦¦^An+lfn41AnhAn_l^---
which may extend to infinity, where An are i?-modules and every /„ is an
i?-homomorphism is called a O-sequence or a complex if every sequence
f f
An+i -3-1 An -A- An_i of three consecutive terms is a 0-sequence while it is
said to be an exact sequence or an acylic complex if every such triplet
is an exact sequence.
We write the zero module simply as 0 and 0 —> A. A —> 0 for any
i?-module A are the obvious maps or morphisms.
Lemma 1.3.1 For R-modules A,B and R-homomorphism f : A —»¦ B
(i) 0 —> A —> B is exact if and only if f is a monomorphism;
(ii) A —>/?—>¦ 0 is exact if and only if f is an epimorphism;
(Hi) 0 —> A —> B —> 0 is exact, if and only if f is an isomorphism.
Proof. Exercise.
Let A be an i?-module. B be a submodule of A. a : B —> A the inclusion
map and /? : A —> A/B be the natural projection i.e. /3(a) = a + B. a ?
© 2003 by CRC Press LLC
A. Then a is a monomorphism. [3 an epimorphism and Im a = B = Ker [3.
Thus the sequence 0^54aA A/B -> 0 is exact.
An exact sequence of the form 0—> A ¦% B —)¦ C —>¦ 0 is called a short
exact sequence.
Let p be any prime and Zv? denote the cyclic group of order p2. Let
p : Zp2 —> Zp2 be the multiplication by p. This is a homomorphism and the
long sequence
- ¦ - —> Zp2 —> Zp2 —> Zp2 —> Zp2 —> • ¦ ¦
is exact. However the sequence
is not exact but. the sequence
0 -> Zp -> ZV2 4 ZP2 -> Zp -> 0
is exact. Decide the unmarked maps in this sequence.
Proposition 1.3.2 For a short exact sequence
A.9) 0->A4/?4f7->0
of R-modules and homomorphisms, the following are equivalent :
(a) there exists a homomorphism a : B —»¦ A such that af = 1a',
(b) there exists a homomorphism C : C —»¦ B such that g C = lc;
(c) Im f is a direct summcmd of B.
Proof. We give a circular proof of this result.
(a) => (c). Suppose that there exists a homomorphism a : B -»¦ A such
that a f = lyt-the identity map from A to A.
Let b G B. Then a f a(b) = a(b) so that. a(b - fa(b)) = 0. Then
b — fa(b) ? Ker a = K (say) and we have b = k + fa(b) for some k ? K.
Thus B = K + Im(f).
Suppose that there is also an element k! ? K and an element a ? A such
that, b = k + fa(b) = k! + f(a). Applying a to both sides of this relation
we get
a(k) +af a(b) = a(k') + a f(a)
or a(b) = af(a) = a.
But then k! = k. Hence every element of B can be uniquely written as
k + f(a) for some k ? K, a ? A. Therefore B = K © Im f and (c) holds.
(c) => (b). Suppose that, there exists a submodule K of B such that. B =
K®Imf.
© 2003 by CRC Press LLC
Let c G C. Then there exists b? B such that, c = g(b). Letb = k + f(a)
for some k G K. a G A. Then c = g(k). If fc' G K is another element such
that c = g(k'). then <?(&' — fc) = 0 which shows that k' — k = f(a') for some
a' ? A. The direct sum property of B shows that k! — k = f(a') = 0 or
that, k! = k. Hence there exists a unique k ? K such that, c = g(k). Define
[3 : C —> B by [3{c) = k. where k ? K is the unique element, such that.
g(k) = c. The map C is an i?-homomorphism and we have
g[3{c) = g(k) = c for every c ? C.
Hence g/3 = lc-the identity map of C.
(b) => (a) Suppose that there exists an i?-homomorphism [3 : C —> B such
that gC = lc-
Let b ? B and suppose that. g(b) = c. Then g(b) = c = g[3{c) so that.
g(b - C{c)) = 0. Then there exists a G A such that, b = C{c) + /(a). If we
also have
/3(c) + f(a) = f3(c') + f(a')., then f3(c> - c) = f(a - a').
Therefore, 0 = gf(a - a') = g/3(c' - c) = c' - c i.e. c' = c. But
then a! = a also. Hence every element, of B can be uniquely written as
C{c) + /(a), c G C, a ? A. Define a map a : B ->¦ A by
a(P(c) + f(a)) = a., c ? C, a ? A.
a is a well defined homomorphism and af = lA- This proves (a), q
Definition 1.3.3 If any one of the three equivalent conditions of Proposi-
Proposition 3.2 is sastisfied. then A.9) is called a split exact sequence or that
the sequence A.9) is said to split.
Corollary 1.3.4 If the exact sequence A.9) splits and a, [3 are as in Propo-
Proposition 3.2, then (i) R = f(A) © /3(C) = f(A) © Kerg ^ A®C and (ii)
a/3 = 0.
1.3.5 Exercises
1. Prov that the sequence 0->A->BA-C->0of R- modules and
homomorphisms is split exact if and only if there exist homomorphisms a :
B^A.,p:C^B satisfying af = 1A, gf = 0, gp = lc, fa + pg = 1B
and a/3 = 0.
2. Let 0^i->B4C->0bean exact sequence of i?-modules and
homomorphisms.
(i) If A, C are finitely generated, then prove that so is B.
(ii) Tf B is finitely generated, prove that C is finitely generated. Is A also
finitely generated ? Justify.
3. Tf 0 -»¦ A -»¦ B -4 F -»¦ 0 is an exact sequence and F is a free
i?-module. prove that, the sequence splits. Is the condition of F being free
necessary ?
© 2003 by CRC Press LLC
4. If 0 —> A —> B -A- C —> 0 is an exact sequence of finite Abelian groups
and orders of A and C are coprime. prove that, the sequence splits.
(Hint : Let O(A) = m; O(C) = n; where O(K) ( as also \K\) denotes for
a group K the order of K. Let r,s ? Z such that mr + ns = 1. Define a
map 7 : C —> B by 7@) = mr b. where g(b) = c).
One of the central idea prevalent in homological algebra is that of di-
diagram chasing. We next consider a couple of simple results of this nature
one of which is embodied in the Five Lemma.
A diagram
A
of i?-modules and homomorphisms is said to be commutative if ga = /3 f
while the diagram
A
is said to be commutative if a f = g.
The idea of commutativity of larger diagrams is understood in an obovious
way.
For example : (i) if / : A —> B is a homomorphism of i?-modules and
L = Ker f, f : A/L —> B is the induced homomorphism i.e. f(a + L) =
/(a), a ? A. then / = fir. where tt : A —> A/L is the natural projection.
Thus the following diagram is commutative
© 2003 by CRC Press LLC
(ii) If M is an i?-module and A,B are submodules of M with A C B.
let 7T : B ->¦ I?/A tt' : M ->¦ M/^4 be the natural projections and i : B/A -»¦
M/A. j : B —$¦ M be the inclusion maps. Then itt = tt'j and the following
diagram is commutative
B
M-
B/A
M/A
Lemma 1.3.6 (Five Lemma) . Consider a commutative diagram
h
At,
0i
B,
BA
04
of R-modules and homomorphisms with exact rows, (i) If ti and ?4 are
epimorphisms and ?5 is a monomorphism then t% is an epimorphism.
(ii) If ti andti are monomorphisms and ti is an epimorphism then t% is a
monomorphism.
Proof, (i) Suppose that, t^, ?4 are epimorphisms and ?5 is a monomor-
monomorphism. Let b?, ? B3. Then G3F3) € B4 and since ?4 is an epimor-
epimorphism. there exists an element aA ? A\ such that 53F3) = t\{a\). Now
^5/4@4) = <M4@4) = 0403F3) = 0 and ?5 is a monomorphism. There-
Therefore. fi(a4) = 0. The upper row being exact, there exists an element
a3 G As such that. /3(a3) = a4. Then 53F3) = ?4@4) = ?4/3(^3) =
3) and so. 53F3 - t3(a3)) = 0. Therefore there exists an element.
© 2003 by CRC Press LLC
hi ? B2 such that. b3 — ?3A23) = g% F2)- The homomorphism ?2 be-
being an epimorphism, there exists an a? ? A2 such that b2 = ?2@2)-
But then b3 - t3(a3) = 32F2) = 52(^2@2)) = E2*2)@2) = ?3/2@2) or
63 = ^3/2(^2) + ?3@3) = ?3@3 + h{ai))- Hence ?3 is an epimorphism.
(ii) Now suppose that ?2, ?4 are monomorphisms and t\ is an epimorphism.
Let <23 ? A3 such that. ?3A23) = 0. Then
0 = 53^3@3) = ?4/3@3)-
?4 being a monomorphism, we have 73@3) = 0 and. therefore, there exists
an element a^ ? A2 such that a3 = 72@2)- But then g^t'iia^) = ?3/2@2) =
?3@3) = 0 and, therefore, there exists an element 61 G Bi such that ?2 (a2) =
<7i(&i). Since ?1 is an epimorphism, there exists ai ? A1: such that ?1@1) =
bi. Then ?2(a2) = 51F1) = 5i^i(ai) = hfi{ai).
Now ?2 being a monomorphism, we have a? = fi(ai) and, hence, a3 =
/2(/i(<2i)) = 0. This completes the proof that ?3 is a monomorphism. q
Combining (i) and (ii) of the lemma, we have
Corollary 1.3.7 Ift2 and ?4 are isomorphisms, ?1 an epimorphism andtc,
a monomorphism, then ?3 is an isomorphism.
Remark 1.3.8 Observe that in the proof of (i) of the five lemma, the maps
tiifi>9i do not play any part while in the proof of (ii) of the lemma ?5, j'4, g^
do not play any part.
Making use of the above observation, we can have as an immediate
consequence of the lemma
Corollary 1.3.9 Consider a commutative diagram
h A h
0
A1
?1
?2
A3
?3
B3
0 BY B2 B3 0
01 02
with exact rows. If any two of ti,t2,t3 are isomorphisms, then so is the
third.
For the proof of this we can think of maps ?0 : 0 —> 0 and ?4 : 0 —> 0
given making the diagram
0
0
?0
?1
?3
?4
B3
© 2003 by CRC Press LLC
commutative. Both to,ti are isomorphisms. When <i,<3 are isomorphisms
then so is ti is the result of Corollary 3.7. Tf t2,t3 are isomorphisms, it
follows from Lemma 3.6 (i) that t\ is an epimorphism. If a\ ? A\ such that
t\{a\) = 0. then 0 = giti(ai) = ?2/1@) and both /i,^2 being monomor-
phisms «i = 0. Hence t\ is a monomorphism and so an isomorphism.
If t\, <2 are isomosphisms, then Lemma 3.6 (ii) shows that ?3 is a monomor-
monomorphism. Also, for any 63 G _B3. there exists 62 G -B2 such that 52(^2) = 63-
Then there exists an a^ ? Ai such that hi = ?2@2)- Therefore
b3 = 52F2) = S2(Ma2)) = E2?2)(a2) = (<3/2)(a2) = t3(f2(a2))
showing that ?3 is an epimorphism. Hence ?3 is an isomorphism.
Proposition 1.3.10 Consider a diagram of R-modules and homomorphisms
M
0
A
B
C
a
with exact row such that C f = 0. Then there exists a unique homomorphism
g : M —»¦ A such that ag = f.
Proof. Exercise.
Corollary 1.3.11 Given a commutative diagram
f n 9
A
R
C
/' 9'
of R-modules and homomorphisms with g f = 0 and lower row exact, there
exists a unique homomorphism a : A —»¦ A' such that fa = (if.
Proof. Here j3 f '¦ A —> B' is a homomorphism such that g' (/? /) =
(9'0)f = G5)/ = 7E/) = 0. Then, by the proposition there exists a
unique homomorphism a : A —»¦ A' such that, fa = ft f. rj
Proposition 1.3.12 Given a diagram
a _ 0
A
B
M
C
0
© 2003 by CRC Press LLC
of R-modules and, homomorphisms with exact row such that fa = 0, then
there exists a unique homomorphism g : C —»¦ M such that f = g/3.
Proof. Let c ? C. The map C being an epimorphism. there exists a
b G B such that /3(b) = c. If b' G B is another element such that /3(b') = c.
then f3(b' — b) = 0 and there exists an element a G A such that, b' — b — a(a).
But then /(&') - /(&) = /(&' - 6) = /a(a) = 0 showing that, the element.
f(b) G M is independent of the choice of the element b G B such that
/3{b) = c. Define g : C ^ M by g(c) = f(b). where 6 G B is such that
Let ci,c2 G C,r G i?. Choose 61,62 G -B such that. 0(bi) = ci,/?F2) = c2 so
that. 5(Cl) = /Fi),5(c2) = /F2). Then
P(h) + P(b2) =Cl+ c2; 0(rh) = r/3(h) =ra
which imply that.
g(ci + c2) = f(h + b2) = f(h) + f(b2) = g(Cl) + g{o2)
= f(rh) = rf(bi) = rg(d).
Therefore g is an i?-homomorphism. That g/3 = f is clear from the defini-
definition of g.
Let h : C —> M be another homomorphism such that, h/3 = f.
Let c G C and choose b ? B such that. C(b) = c. Then g(c) = f(b) =
(hP)(b) = h(P(b)) = h(c) which shows that, g = h. n
Corollary 1.3.13 Given a commutative diagram
a
r '
of R-modules and homomorphisms with g'f = 0 and the upper row exact,
then there exists a unique R-homomorphism 7 : C —»¦ C" such that 7,<? =
Proof g'P : B —> C is a homomorphism such that. (g'P)f = g'ifi /) =
g'(f'a) = (g'f')a = 0. Therefore, by the proposition there exists a unique
homomorphism 7 : C —> C such that g'/3 = 7,9. q
1.3.14 Exercises
Let
© 2003 by CRC Press LLC
Al
A2
A3
i?2
-B3
01
02
be a commutative diagram of i?,-modules and homomorphisms with exact
rows.
(i) If ti,tz,g\ are monomorphisms. prove that, ti is a monomorphism.
(ii) If ti,ts, fi axe epimorphisms. prove that, ti is also an epimorphism.
(iii) If <i, <3 are isomorphisms. <?i is a monomorphism and fc is an epimor-
epimorphism. prove that ti is an isomorphism.
1.4 Homomorphisms
1.4.1 Let A,B be -ft-modules. Let HomR(A,B) denote the set of all R-
homomorphisms from A to B. For /, g G Homn(A, B) define / + # : A -»¦ /?
by
(f + 9)(a)=f(a)+g(a):
For a, ai,a2 G A, r G R,
f(a1 + a2) + g(ai
(f(a2) + g(a2)) =
f(a2)+g(a1)+g(a2) =
and
(f + g)(ra) = f(ra)+g(ra) = rf(a) + rg(a) = r(f(a)+g(a)) = r(f + g)(a)
Hence / + g G HomR(A, B).
The map 0 : A —> B given by 0(a) = 0 for every a G A is an i?.-
homomorphism and for every / G Homn(A, B). f + 0 = / = 0 + /.
For / G HomR(A, B) define g : A -> B by
0(a) = ~/(a):
If a, ai, <22 G A r G R.
g(ai+a2) = -f(ai+a2) = -
and
= -f(ai)-f(a2) = g(ai)+g(a2)
= -r/(a) = r(-f(a)) = rg{a).
5 = 0 = 5 + /is clear.
Thus g G HomR(A, B). That / + 5 = 0 = 5
If f,g,h? Homn(A, B) and a G A. then
((/ + s) + *0(a) = (f + g)(a) + h(a) = (f(a)+g(a)) + h(a) = f(a) + (g(a)
h(a)) = /(a) + E + ft) (a) = (f + (g + h))(a)
© 2003 by CRC Press LLC
and we have (/ + g) + h = f + (g + h) i.e. the additive composition in
Homn(A,B) satisfies the associative law.
Again . for /, g G HomR(A, B), a € A,
(/ + g) {a) = f(a) + g(a) = g(a) + f(a) = (g + f)(a).
Therefore / + g = g + f
Hence Homn(A,B) is an Abelian group.
Tn general Homn(A,B) is not an R-module . However, we have the
following .
Proposition 1.4.2 If R is a commutative ring and A, B are R-modules,
then Homn(A,B) is an R-module.
Proof. For r G R, f G HomR(A, B) define r / : A -> B by
(rf)(a)=rf(a)., a & A.
For a, ai, <ii G A. s G R.
a2) = r/(ai + a2) = r(/(<n) + /(a2)) = r/(<n) + r/(a2) =
(r/)(a2)
and
(r/)M = rf(sa) = r(sf(a)) = (rs)f(a) = (sr)f(a) = s(rf(a)) = s(rf)(a).
Therefore rf G HomR(A,B).
It is fairly easy to check that then the Abelian group Honin(A, B) becomes
an i?-module. q
In the case when the ring R is not necessarily commutative we can have
the following
Proposition 1.4.3 For any left R-module A, Homn{R,A) is again a left
R-module.
Proof. For r G R, f G HomR{R,A) define rf : R. -> A by (rf)(s) =
f{sr). s G R. It is almost, trivial that. (r/)(si + s2) = (r/)(si) + (r/)(s2)
for Si,S2 G -ff. Let s, Si G i?. Then
(r/)(ssi) = /((ssi)r) = /(s(sir)) = s f{slr) = s(r/)(si).
Thus r / is an i?-homomorphism.
Thatr(/i + /2) = r fi+r f2,r1(r2f) = (rir2)/and 1/ = /forr,ri,r2 G i?;
/i>/2)/ G Homn{R,A) are easy to check. This completes the proof that
Homn{R,A) is a left i?-module. q
Theorem 1.4.4 For any R-module A, Homn(R,A) = A as R-modules.
© 2003 by CRC Press LLC
Proof. Define a map 0 : HomR(R,A) -> A by 0(f) = f(l), 1 the
identity of R. f G HomR(R,A).
The map (9 is a homomorphism of i?,-modules.
Tf 0(f) = 0, for an / G HomR(R,A), then /(I) = 0 and for any r G
R. f(r) = /(r.l) = r/(l) = r.O = 0 i.e. / = 0. Hence 0 is one-one .
Let a G A. Define / : i? -> A by /(r) =ro. r G -ft.
The map / is an i?-homomorphism and 0(f) = /(I) = a. Thus 0 is an
epimorphism and hence an isomorphism, rj
Let {Mi}i?i be a family of i?-modules. ffi ?,e/ -^j the direct sum and
nie/Mi the direct product of the family with a.j : Mj ->¦ © Xlie/ -^»> j?^
the natural injection and itj : HiMi —> Mj. j G /. the natural projection.
Theorem 1.4.5 For any R-module A,
(i) UiHomniMi, A) =s HomR{® Y,Ml,A)
(ii) UiHorriRiA^Mi) ^ HomR^IUMi)
When R is commutative, the isomorphisms in (i) and (ii) above are R-
isomorphisms.
Proof, (i) Define maps 0 : HomR(® Yl Mi, A) ->¦ IIji?omfl(Mj, A) and
i, A) -> HomR(® ? Mh A) by
and
«^((ft)) = 5. 9i e HomR{MuA)., i G /,
where 5((^)) = ?• 5i(^): fe) G © Eie/ Mi-
The maps ^ and cf> are homomorphisms of Abelian groups. Let
/ G HomR(®^2Mi,A). Then
(<t>9)(f) = <j>@(f)) = H(f*i)) = d>(Gl)) = 7 (say),
where ft = fai, i G T. For any (xt) G
showing that / = /. Therefore 06»(/) = / for every / G HomR{® ? Mi;
Hence <f>0 = identity and so. 0 is a monomorphism.
Let ft G HomR(Mh A), i G T. Define 5 : © ? Mt -> A by
For Xj G Mj. 7 G /. let. ckj(xj) = (yj): so that, t/j = Xj and yj = 0 for j 7^ 7
and we have
(gaj)fxj) = o(aj(x,-)) = <?((Vi)) = >^ fi(Vi) = fi(xj).
.7
© 2003 by CRC Press LLC
Therefore. gat = ft for every i G / so that. 6(g) = (gai) = (/,) showing
that 9 is an epimorphism and. hence, an isomorphism . Tf the ring B, is
commutative, both Homn{® ^2 Mi, A) and HHomn(Mi, A) are i?-modules
and it is clear that 0 is an i?-homomorphism and, hence, an i?-isomorphism.
(ii) Define maps 6 : Homn(A, IIjMj) -»¦ UiHomR(A, Mi).
<j>: UiHomR(A,Mi) -> HomR(A,UiMi) by
0(f) = fa/), / G HamH^UiMi), ct>{{fi)) = f, fi G HamR(A,Mi),
where /(a) = (/i(a)), a G A.
^ and (f> are homomorphisms of Abelian groups. If / G HomR(A,IliMi).
0(f) = M) and mU) = mi)) = <Knf) = 7-, where/(a) = (Gri/)(a))'
= (GrJ(a))) = /(a) for every a G A.
Therefore, <f>8 = identity.
Consider (/,) G UiHomR(A, Mt) with fi G HomR(A,Mi), i G T. By
definition <j>((fi)) = f, where
/(a) = (^(a)), a G A
Then fl@((/i))) = 0(/) = (ttJ) and for any a G A i G /, GrJ)(a) =
Ti(/(a)) = /i(a) so that nj = fi and ^((/i)) = (ttJ) = (/<). Hence 9<j> =
identity. Therefore 0 is an isomorphism with <f> as its inverse isomorphism.
When the ring B, is commutative both HiHoniji(A, M{) and
HomR(A,Ti.iMi) are i?-modules and 0 is trivally an i?-homomorphism. rj
We need a lot. more properties of Homn(—, —) and we shall come back
to these after we have introduced what we call functors.
When A, B are Abelian groups (and so Z-modules), we write Hom(A,B)
for Hornz {A, B).
1.4.6 Exercises
1. If Q is the additive group of rational numbers and Z the additive
group of integers, describe
(a) Hom(Q,Q/Z);
(b)Hom(Q/Z,Q/Z);
(c)Hom(Q/Z,Q).
2. If A is a torsion-free , divisible Abelian group and B any Abelian
group, prove that Hom(A,B) is a torsion-free, divisible Abelian group.
3. If A is a torsion Abelian group and B a torsion-free Abelian. group,
prove that Hom(A, B) = 0
4- If A is a left R-module, define r f : B, —> A, for r G R. / G
Homz(R,A), by (r f)(s) = f(sr). s G R. Prove that under this action
Honiz (-R, A) is a left R-module.
© 2003 by CRC Press LLC
1.5 Tensor Product of Modules
In this section we consider the construction of an Abelian group from a
given pair of modules one of which is a right R-module and the other a left
R-module.
Definition 1.5.1 Let M be a right i?-module, AT a left i?-module and G
an Abelian group. A map / : M x TV —> G is called i?-biadditive if
(i) /(mi + m2,n) = f(mi,n) + f(m2,n),
(ii) f(m, n1+n2) = f(m, ni) + f(m, n2).,
(iii) f(mr,n) = f{m,rn)
for all m, mi, 7712 ? M,n,ni,n2 € TV, r € R..
Definition 1.5.2 Let M be a right -R-module. N a left i?-module. By
tensor product of M by AT over B. we mean an Abelian group M ®r TV
and i?-biadditive map h:MxN—>M®f{N such that, for any Abelian
group A and any i?-biadditive map / : M x TV —> A. there exists a unique
homomorphism g : M ®/j TV —> A of Abelian groups which makes the
diagram
M x TV —*¦ M®RN
commutative i.e. gh = f.
Proposition 1.5.3 Any two tensor products of M by N over R are iso-
morphic.
Proof. Let A and B be two tensor products of a right i?-module M by a
left i?-module TV. Then there also exist. i?-biadditive maps hi : M x TV —> A
and hi : M x AT —»¦ B. Since (A, hi) is a tensor product of M by TV and hi :
M x AT —> B is an i?-biadditive map, there exists a (unique) homomorphism
/ : A -»¦ B such that f hi = h2. Again (B,h2) being a tensor product of
M by TV, there exists a (unique) homomorphism g : B —»¦ A such that.
ghi = hi. But then we have hi = g(f hi) = (g f)hi and hi = (f g)hi.
Also the identity homomorphism 1a '¦ A —> A and 1b : B —> B have the
property that hi = lAhi and hi = lsh2.
The uniqueness of the homomorphism in the definition of tensor product
then shows that, g f = 1a and f g = 1B showing that / is an isomorphism
with g as its inverse, q
1.5.4 We next consider the question of existence of tensor product of a
right i?-module by a left R-module.
© 2003 by CRC Press LLC
Let M be a right i?-module and TV be a left i?-module. Let Z(M, N)
be the free Z-module freely generated by the set M x Af = {{m,n)\m G
M,n G N}. Let B{M,N) be the submodule of Z{M,N) generated by all
elements of the form
(a) (mi + m2,n) - (mi,n) - (m2,n).
(b) (m,ni + n2) - (m,ni) - (m,7T,2);
(c) (mr,n) - (m,rn)
for all m,mi,m2 ? -W; n,ri\,ni G N and r G B.
Denote the quotient Z-module Z(M,N)/B(M,N) by M ®R N.
To simplify our notation, we write m $5 n for the element (m,n) +
B(M,N) of M ®n N. Since for m1)m2 G M. n ? N, (mi + m2,n) -
(mi,n)-(m2,n) G B{M,N), (m
0,i.e. (mi+m2,n)+JB(M,7V) =
or
A.10) (mi + m2) ®n = mi ®n + m2 $5 n.
Similarly
A.11) m $5 (rii + n2) = m ® ri\ + m $5 n2.
and
A.12) (m r) ® n = m & (m)
for all m G M. n,ni,n2 G TV. r G i?..
As a consequence of these properties, we also ha,ve 08n = m®0 = 0
for all m ? M. n ? N and (-m) &n = m ® (-n) = — (m (8 n) for all
m G M. n G N.
Define a map h : M x N ^ M ®R N by
h{m, n) = m<E)n. m ? M. n G N.
It is clear from A.10). A-11) and A.12) that, h is an i?-biadditive map.
Let A be an Abelian group and /:MxJV->Abean i?-biadditive map.
Z(M, N) being a free Z-module on M x TV. extend the map / to Z-module
homomorphism g : Z(M, N) —> A.
Explicitly
g(^2Xi(mi,ni)) = ^Xif(mi,ni),Xt G Z, rrii G M, nt G N.
For mi,m2 G M. n G AT
) - (m2,n)) = /((mi + m2,n)) -
/((m2,n)) = /(mi,n) + f(ni2,n) - f(mi,n) - f(rri2,ri) = 0. as / is biad-
ditive.
Similarly
g((m, m + n2) - (m, m) - (m, n2)) = 0. j((mr, n) - (m, rn)) = 0
for all m G M. n,rii,n2 G TV. r G R. Therefore g vanishes on B(M,N)
and. therefore, it. induces a homomorphism
© 2003 by CRC Press LLC
g:Z(M,N)/B(M,N) -> A,
~g(m <S) n) = f(m, n). m G M, n G TV.
i.e. gh = f.
Let <?! : Z(M,N)/B(M,N) —> A be another homomorphism such that
«7i/i = /. For any element. ^A^m* ® ni) G Z(M,N)/B(M,N),\i G
Z, m; G M,m G TV.
showing that gx = ~g.
This proves that M ®rN with i?-biadditive map h : M x /V -> M ®R N
given by h(m, n) = m®n, m ? M, n G TV. is the tensor product of M by
TV over R.
Remark 1.5.5 From the properties A.10) and A-11) it follows that for
any integer k. positive, negative or zero and m G M, n G N,
k(m(?fri) = (km) ®n = m & (kn).
Therefore an arbitrary element, of M ®r N is a finite sum of the form
^nii® rii, rrii ? M. rii ? N.
As is clear from the definition tensor product of i?-modules is an Abelian
group. However, we can make, it. into an i?-module provided R is a commu-
commutative ring. Recall that in this case every left i?-module is a right i?-module
and vise versa.
Theorem 1.5.6 If R is a commutative ring andM,N are R-modules, then.
M ®r N is an R-module.
Proof. Let r ? R. Define a map r* : M x TV ->¦ M ®R N by
r*(m,n) = (mr) ® n = m ® (r n). m ? M. n G N.
It is fairly clear that.
r*(mi + m2, n) = r*(mi,n) + r*(m2,n).
r* G71,77,1 + n2) = r* (m, 77,1) + r*(m,7i2);
© 2003 by CRC Press LLC
for all m,mi,77i2 ? M and n,7ii,ri2 G TV.
For m G M,n G TV, s G i?, r*(ms,n) = (ms)r $5 n = m(sr) 8n =
m(r s) 0 n = (m r)s ®n= (mr)8(sn) = r*(m, s n).
as R is commutative Therefore r* is i?-biadditive. Then there exists a
unique homomorphism fr : M $§_r TV —> M $§_r TV such that, the diagram
M x TV —»¦ M ®RN
\
r*
M ®R TV
where h(m, n) = m $5 n, m G M, n G TV, is commutative.
Define
= ^2Tniig)rni,mi ? M, rii ? N.
It is fairly easy to check that then M Cr N becomes an i?-module. q
Proposition 1.5.7 For every left R-module M, R®rM is a left R-module
and for any right R-module N, N $§_r R is a right R-module.
Proof. Consider a right i?-module AT. For r ? R. define a homomor-
homomorphism r* : Z{N, R) -> TV ®R R by
r*((i,s))=i8(sr). x G TV, s G R.
r* clearly vanishes on the generators of B(N, R) and, therefore, on B(N, R).
Therefore, r* induces a homomorphism r* : TV ®_r R —> TV ®_r R. where
r*(i®s) = i8(sr): x G TV, s ? R.
Define the right action of R. on TV ®_r i? by
zr = r*(z), 2r G TV ®rR and r G R.
That (zi + ^2)r = z-\r + z?y., z.\ = z for z,zi,Z2 ? AT $§/j R. r ? R. and
1 the identity of i? are clear. Let r\,r2 ? R- For any x ? N,s ? R,
(x $5 s)(Vi + r2-) = (r\ + r2)*(x g) s) = i ® s(ri + r2-) = x $5 (sri + sr2) =
x ® {s n) + x & (s T2) = r*(x $5 s) + rj(x <g> s) = (x ® s)ri + (x $5 s)r2- Also
(x $5 s)(ri f2) = x $5 s(ri r2) = x $5 (s ri)f2 = (i®s ri)f2 = ((x $5 s)n)r2-
© 2003 by CRC Press LLC
These imply that.
z{r\ + r2) = zr\ + zr2 and (zri)r2 = z(ri r2)
for every z ? N ®R R and ri, r2 G R. Hence TV ®R R is a right i?-module.
That R (&r M becomes a left i?-module can be proved similarly, q
Theorem 1.5.8 For every R-module M, R (X M = M as left R-modules
and for every right R-module N, N Cr R= N as right R-modules.
Proof. Let M be a left i?-module. Define a map a : M -»¦ R ®R M by
a(m) = l®m, m G M.
a is clearly an onto homomorphism of Abelian groups (any element of
is of the form Yl n^mt = Yl 1 ri®rrii = Yl l^i mi = 1®Z! ri mi =
, where m = Yrimi ^ M). Also a(r m) = l®rm = r®m = r.l®m =
r(l ®m)= ra(m). Thus a is an i?-homomorphism.
On the other hand, define a map ft : R. x M -»¦ Af by
P(r,m) = rm.r € R, m ? M.
For r,s ? R. m, mi, m2 G M.
/?(r + s, m) = (r + s)m = rm + sm = f3(r, m) + C(s, m).
P(r,mi + m2) = r(mi + m2) =rm-\ +rm,2= P(r,mi) + P(r, m2);
/J(r s, m) = (r s)m = r(s m) = f3(r, s m).
Thus P is biadditive and. so. induces a homomorphism ft : R ®R M ->¦ M
such that.
0(r ®m) = rm, r G R, m G M.
It is clear that. Pa = \m and a/3 = 1_r®rm- Hence a is an i?-isomorphism
with j3 as its inverse.
That. TV ®r R = N for any right i?-module TV follows similarly, q
1.5.9 Let A, M be right -R-modules. B. N be left i?-modules and / : A ->
M and <? : i? —> N be i?-homomorphisms.
Define a map 9 : A x B ^ M ®R N by
6>(a,6) = /(a)<g>g(&); a?A,b?B.
For tt,tti,a2e A b ? B, r ? R, we have
%i + a2, 6) = /(ai + a2) «) 3F) = (/(ai) + /(a2)) ® g(b) = /(ai) (8) flF) +
f{a2)®g{b)=0{al,b)+0{a2,b):,
0{ar,b) = f{ar) ® flF) = /(a)r ® g(b) = /(a) ® r<?F) = /(a) ® fl(r6) =
9(a,rb):
That ^(a, 6i + b2) = 9{a, bi) + 9{a, b2) for all a ? A, bi, b2 ? B. follows on
similar lines. Thus 0 is i?-biadditive and. therefore, induces a homomor-
homomorphism : A ®r B -»¦ M ®r N in which a $5 b. a ? A,b ? B, gets mapped
onto /(a) $5 <?(&)¦ This homomorphism induced by 9 is denoted hy f ® g.
© 2003 by CRC Press LLC
Now we are in a position to prove that, tensor product commutes with
direct sums.
Theorem 1.5.10 (i) If M is a right R-module and {A^Lc/ is a family of
left R-modules, then. M ®R @ ? .?/ TV,) ?z ffi ]T\ M ®R Nt.
(ii) If N is a left R-module and {M{}i^j is a family of right R-modules,
then (®ElMi)®RN^®Yi(Mi®RN).
Proof, (i) We prove (ii) and (i) shall follow on similar lines. For every
j G /, let aj : Mj -»¦ ffi Y Mi be the natural injection and itj : ffi Yiel Mi ->¦
Mj be the natural projection. Then
1 if i = j
0 if i ^ j
Also Yi(aini)(a) = a f°r every a G ffi Y,iei Mi-
For every j G /. the maps a, and ttj induce homomorphisms
i
itj ® 1 : (ffi Y^ Mi) ® N -> Mj ®RN,
Let
9 : © ^2(Mi ®R N) -> (© ^2 Mi)
i i
(j> : (© J2 Mi) ®R N -* ©
i
be the homomorphisms induced by {oj $5 1} and {ttj $5 1} respectively.
Then for a G © Yl Mi, n G N, <f>(a <g> n) = (^(a) <g> n) and for x = (xj) G
©E(^^-R^): ^ ?Mi®RN, 6{X) = Yti{Ui®l){Xi).
For x = (xj) G ffi Y,i(Mi ® ^)- tne ?t/l component of «?0(x) is
((tTjCKj $5 1)(xj) = A $5 1)(xj) = Xj =jth component of x. as 7r7ai = 0 for
* t4 i-
Therefore tf)9(x) = x and so. <f>0 = identity map.
For a G ®Y,Mi; n G AT.
^(a <g> n) = 6»(GTi(a) <g> n)) = Yi(ai ® 1)Gri(a) ® n) = Ej^i^iW ® n) =
(Ei cti7Tj)(a) ®n = d®n.
which shows that B(j> is also the identity map . Hence 0 is an isomorphism
with (f> as its inverse, q
Remark 1.5.11 If R is a commutative ring then ffi ^2(Mi Cr N) is an
-R-module and so is (®Y,Mi) ®R N- For a G © Y.Mi; n G AT. r G i?.
<j)(r(a®n)) = <j)((ar)®n) = GTj(ar)<g>n) = ((^(a) r)<g>n) = (rGTj(a)(g)n)) =
rGTj(a) ®n) = r^(a $5 n)
which shows that <f> is an i?-homomorphism. Therefore, in this case the
isomorphisms of Theorem 5.10 are i?-isomorphisms.
© 2003 by CRC Press LLC
Definition 1.5.12 Let Rop be the set. R with the additive composition the
same as in R. For r, s G Rop. define multiplication by r o s = s r where on
the right hand side is the multiplication as in R. Then Rop again becomes
a ring called the opposite ring of R.
Let A be a left i?-module. For a ? A, r ? Rop define a o r = ra.
This action of Rop on A makes it a right i?op-module. Similarly, a right
i?-module can be made into a left i?op-module.
1.5.13 Exercises
1. Prove that for any left i?-module M. the left i?-modules R ®r M
and M are isomorphic.
2. Tf A is a right R-module and R a left R-module. then A ®R B =
B ®Rop A.
1.6 Direct and Inverse Limits
Definition 1.6.1 A set S is called a directed set if there is a relation <
defined on S such that: (i) < is reflexive, (ii) < is transitive and (iii) for
every pair a,/3 ? S. there exists a 7 ? S such that a < 7 and C < 7. (We
will also write C > a for a < C).
Definition 1.6.2 A direct system of sets {X, tt) over a directed set S is
a function which attaches to each a ? S a set I", and. to each pair a, ft
with a < /3 in S. a map n^ : Xa —> X$ such that, for each a ? S.
tt" = identity map from Xa to Xa. and for a < /3 < 7 in S.
Definition 1.6.3 Let {M, n}s be a direct system over a directed set S.
such that for each a ? S. Ma is an i?-module and for every a < /3 in
S. irP, : Ma —$¦ M@ is an i?-homomorphism. Let Q be the submodule of
® ^2aes Ma generated by all elements of the type ir^x — x,xG Ma. a, ft ?
S and a < /3. The quotient module Q)^MajQ is called direct limit
of the direct system {M, n}s and is denoted by lim^{M, n}s- Observe
that we are here identifying Ma with its canonical image in the direct
sum © Yl Ma- The natural projection © Yl Ma ~^ lim^{M, tt)s restricted
to the submodule Ma of © Yl Ma defines homomorphism ?r« : Ma ->
,n}s called projection and given by na(x) = x + Q. x ? Ma.
We next consider some simple properties of the homomorphisms ira and
Let a,C G 5 with a < C and x G Ma. Then na(x) = x + Q =
x - -k^x + ir^x + Q = it^x + Q = irptn^x) = irpirP(x). We thus have
© 2003 by CRC Press LLC
Lemma 1.6.4 If a < E in S, then, tt/jtt^, = TT
Let u G lim^{M,7r}s. Then u = a + Q with a G ®Y,Ma-
element a is sum of a finite number of elements each coming from some
Ma. Suppose a = ai + a-i + ¦ ¦ ¦ + an. where at G Mai. Choose an a G S
such that, a > ai for every i, 1 < i < n. Then
u = a + Q = a\ + a2 + ¦¦¦¦ + an + Q
= ai - Tr^ai + a2 - 7r^2a2 -\ h an - 7r^nan
where 6 = tt^Qi + .... + 7r«nan G Ma.
This proves
Lemma 1.6.5 If u ? lim^{M, n}s, then there exists an a G S and an
xa G Ma such that 7ra(xa) = u.
Lemma 1.6.6 If xa G Ma and 7ra(xa) = 0, then there exists ft > a such.
P(xa) =0.
Proof. Let x G Ma such that na(x) = 0. Then x G Q and so.
where a,/3, • • •, A are all distinct, o^ are all distinct, and so are all /3j, ¦ ¦ ¦.
and all A&. There being only a finite number of o^ ,/?,-,¦•¦ Afe. the number of
elements of S appearing in the above equation is finite. Choose 7 G S such
that 7 > all the elements of S appearing in this equation. Observe that
A.13) is an equation in ®^Ma and. so. comparing components on the
two sides, we get equations in Mx for different A's in which the equation
corresponding to A = a has x on the left hand side while every other
equation has 0 on the left hand. Applying ttJ to both the sides of the
equation corresponding to A for every A and adding the resulting equations
we get
k
0-D
© 2003 by CRC Press LLC
Remark 1.6.7 If U\, ¦ ¦ ¦, un are a finite number of elements of
lim^{M,n}s, then there exist oii G S, and Xi G Mai, 1 <i < n, such that
Ui = nai(xi). Choose a > o^. 1 < i < n. Then Ui = 7ra7r". (x,) = na(yi),
where yi = tt™. (x^) G Ma for i = 1, 2, • • •, n.
1.6.8 Let M be an i?-module. Let 5 be the set of all finite subsets of M.
For a ? S. let Ma be the submodule of M generated by the subset a of
M. For a,0 ? S. say that a < j3 if Ma is a submodule of Af3 and take
tt^ : Ma —> Ml3 to be the inclusion map. The relation < defined on S is
clearly reflexive and transitive and for a,/3 ? S. we can choose 7 = a U ft
and we get a < 7, @ < 7. Thus S with the relation < defined on it is a
directed set. Also {M, n}s is then a direct system of i?-modules and we
can talk of the direct limit lim^{M,n}s = (B^2MajQ where Q is the
submodule of © Yl Ma generated by elements of the form ir^x — x. where
a,/3 G S with a < ft and x G M". Define a map 6 : M -»¦ lim^{M, ir}s as
follows :
Let x G M. Then there exists a G S such that x G Ma. Let /3 & S
be another element such that x G Af3. Choose 7 G 5 with a < 7,/J < 7.
Then x G M7 as well and 7ra(x) = 7T77r2(x) = ir7(ir1(x) — x) + ?r7(x) =
tt7(x) = 7T77r^(x) = 7r^(x) showing that na(x) is independent of the choice
ofaeS1 for which x G Ma. Set 6»(x) = 7ra(x).
It is fairly easy to see that 6 is an i?-epimorphism. Tf x G M such that
#(x) = 0. then na(x) = 0 for an a G S such that x G Ma. From Lemma
6.6 it. follows that, there exists a ft > a such that tt^(x) = 0 and each ?r^
being a monomorphism x = 0. Therefore 0 is a monomorphism and. hence,
an isomorphism. Identifying x G M with #(x). we can have the following.
Proposition 1.6.9 Every module is direct limit of its finitely generated
submodules.
Next we consider an axiomatic description of direct limit-
Definition 1.6.10 Given a direct family {Ma,ir}s of i?-modules. an R-
module M together with homomorphisms TTn : Ma —> M is called direct,
limit, of the family if
(a) TTn = TTpTT^, for every a < /3;
(b) when /V is another i?-module with a family of i?-homomorphisms
Xa : Ma —> N such that Xa = A^tt^ for every a < /3 then there exists a
unique i?-homomorphism A : M —>¦ AT such that A?ra = Xa for every a G S.
As in the case of any axiomatic definition, we now need to prove that direct,
limit of direct family of modules always exists.
1.6.11 Let {Ma,n}s be a direct family of i?-modules and homomor-
homomorphisms over a directed set 5. Let M = © Yl Ma/Q where Q is the submod-
submodule of © Yl Ma generated by elements of the form tt^x-x. x G Ma, a < /3.
© 2003 by CRC Press LLC
For a ? S. let. irn : Ma —> M be the homomorphism defined by na(x) =
x + Q. x ? Ma. Then na. n^ satisfy the properties as mentioned in Lem-
Lemmas 6.4-6.6. Let AT be an i?-module and for every a ? S. let Xa : Ma ->¦ N
be a homomorphism such that Xa = A^tt^ for every a < /3 in S.
If u ? M. there exists an x ? Ma such that u = na(x). Let y ? M@ be
another element, such that, u = 7rp{y). Choose 7 ? S such that. a,/3 < 7.
Then ir7irl(x) = 7ra(x) = u = n0(y) = ^^}{y) so that ir7{irZ(x) - ^(y)}
= 0.
But then there exists S > 7 such that TT^ijT^x — n^y) = 0 which implies
that. n6a(x) = nsp(y). Therefore Xa(x) = Xsnsa(x) = Xsnsfi(y) = X0(y). Thus
Xa(x) is independent of the choice of x ? Ma such that, u = -Ka(x).
Define A : M -> TV by
X(u) = Xa(x).
where x ? Ma with u = na(x).
Let u,v ? M and x ? Ma. y ? M& such that u = na(x),v = it
Choose 7 ? S such that. a,/3 < 7- Then u = 7T77r2(x) and v =
Therefore
U + V = 7T77r2(x) + 7T77r^(y) = 7T7Gr2(x) + 7
and
= Xa (x) + Xp (y) = X(u) + X(v).
Also if r ? R. then ru = rna(x) = na(rx) and. therefore.
X(ru) = Xa(rx) = rXa(x) = rX(u).
This proves that. A is an i?-homomorphism such that. X~Kn = Xn for every
a ? S.
Let 11 : M —> N be another i?-homomorphism with jU7ra = Xa for ev-
every a ? S. If u ? M, let u = na(x), x ? Ma for some a ? S. Then
X(u) = Xa(x) = iina(x) = fj,(u) which implies that 11 = X.
We have thus proved.
Proposition 1.6.12 The m.odule M = ®YlMa/Q is direct limit of the
direct family {Ma,n}s in the sense of Definition 6.10.
Proposition 1.6.13 Let {Ma,n}s be a direct family of R-modules and
homomorphisms over a directed set S. Let M with homomorphisms na :
Ma -»¦ M, a ? S and N with homomorphisms Xa : Ma ->¦ N. a ? S, be two
direct limits of the given direct family. Then there exists an isomorphism
9 : M —»¦ TV such that 6na = Xn for every a ? S.
© 2003 by CRC Press LLC
Proof. Since M together with homomorphisms TTn : Ma —> M. a ? S.
is a direct limit of the given family, there exists a unique homomorphism 6 :
M —> N such that 9na = Aa for every a G S. Also TV with homomorphisms
Xa : Ma —> N. a ? S. is a direct limit of the given family. Therefore,
there exists a unique homomorphism <j> : N —> M such that. (f>Xa = -Kn for
every a ? S. Then (f>8na = ?!"„ for every a ? S. Also the identity map
1 : M —> M satisfies l.na = na for every a ? S. Then by the uniqueness
of the homomorphism in (b) of the definition 6.10. it follows that (f>8 = 1.
Similarly B(j> = 1 and 0 is an isomorphism with <j> as its inverse, q
1.6.14 There is yet another description of direct limit, which we now de-
describe.
Consider a direct family \Ma,n}s of i?-modules over a directed set S.
Let X be the disjoint union UaGS Ma. In X define a relation ' ~' as follows
If x, y G X. then there exist a,0 G S such that x G Ma. y G Af3. Say
that x ~ y if there exists a 7 > a,/3 such that n^x = njy. The relation
' ~' is clearly reflexive and symmetric. Let x,y,z G X such that x ~ y and
y ~ 2;. Suppose that, x G M°. y G M@ and 2; G M7 for some a,/3,7 G 5.
Then there exist. 8,/j, ? S such that. nsax = niy and n^y = ni^z. Choose
v G S such that i/> 5,fi. Then tt^z = 7r^7r*x = n^n^y = n^y = n^y =
tt^tt^z = n"z showing that x ~ 2; and ' ~' is an equivalence relation. For
auiel. let. [z] denote the equivalence class to which z belongs and let.
M denote the set of all equivalence classes into which X is partitioned by
the relation ' ~'. Observe that, for any z G Ma and ft > a. tt^x ~ z so
that [z] = [tt^x]. Let x,y ? X. Suppose that z G M°. y G ^^ f°r some
a,/3 ? S and choose 7 > a. C in S. Define
In view of the observation nmde above the element, [n^x + nly] is indepen-
independent of the choice of 7 > a,p. If z G Ma. y ? M&, z G M7. i G M5 such
that z ~ 2. y ~ t. there exist n > a. 7. 1/ > /3, S in S such that tt^x = ?r^2:
and Tr^y = Tr^t. Choose A > //, z/ in 5. Then
[z] + [t] = [n^z + n?t] = [nffiz + n^t]
which proves that the sum defined in M is independent of the choice of
representatives of the elements of M. For z G Ma. r G R. define r[x] =
[rx]. The maps ?r^ being R-homomorphisms. the scalar product is well
defined. It is fairly easy to see that. M becomes a left i?-module.
For any a G 5. define nn : Ma -> M by ?ra(x) = [x],x G Ma. The
maps TTn are i?-homomorphisms.
© 2003 by CRC Press LLC
Theorem 1.6.15 The module M together with the R-homomorphisms ira :
Ma —> M defined above is the direct limit of the direct family {Ma,7r}s of
R-modules in the sense of definition 6.10.
Proof. Exercise.
1.6.16 Dual to the concept of direct limit, there is the concept of inverse
limit which we now study.
A non-empty set S with a binary relation '<' (or '>') defined on it.
is called a quasi-ordered set if the relation is reflexive and transitive.
Let S be a quasi-ordered set. By an inverse system of (left) iZ-modules
indexed by the set S we mean a family of modules {Ma}a?s together
with iZ-homomorphisms </>^ : M@ —> Ma whenever a < /3 such that (i)
<f)% : Ma -> Ma is the identity map for every a ? S: and (ii) <fa<f& = <pa
whenever a < /3 < 7 in S.
Definition 1.6.17 Let {Ma, </>^}5 be an inverse system of i?-modules in-
indexed by a quasi-ordered set S. An i?-module M together with i?-homomor-
phisms <pa : M —> Ma for every a ? S is called an inverse limit of the in-
inverse system if (i) <fta = 4>P,(f>j3 for every a < ft: and (ii) for every iZ-module
TV and family of i?-homomorphisms tpa : N —> Ma for every a ? S satis-
satisfying il)a = 4>a'tl)ll whenever a < C. there exists a unique iZ-homomorphism
9 : N -> M such that. 4>a9 = tpa for every a ? S.
Let {Ma, 0^}s be an inverse system of modules indexed by S and let.
(a) M with (f>a: M -> Ma for every a ? S: (b) TV with ipa : N -> Ma for
every a ? S: be two inverse limits of the family. Then there exist, unique
homomorphisms 9 : N -»¦ M and 0 : M ->¦ AT such that <\>a9 = ipa and
tpa(j) = 4>a f°r every a ? S. For any a ? S. 4>a9<p = <pa and tpa(j)9 = tpa.
Also the identity maps 1m : M ->¦ M and ljv : A^ ->¦ AT are such that
4>a^-M = 4>a anfi ipa^-N = ipa- By the uniqueness of the homomorphism
as required in the definition of inverse limit, it. follows that. 9cf> = 1m and
4>9 = ljv- Hence 9 and cf> are isomorphisms with each as inverse of the
other. Hence M = N. We have thus proved
Theorem 1.6.18 Inverse limit of an inverse system of modules, if it exists,
is unique upto isomorphism.
Again, let. {Ma,(p^}s be an inverse system of modules indexed by
S. Consider the direct product UaesMa and for every ft ? S. let p@ :
WMa -> /Vf3 be the natural projection. Let M = {(xa) ? nMa|0^(a;^) =
xa for every a < /?}. The subset M is a submodule of WMa. For
a ? S. let. (j)a : M -»¦ Ma be the restriction of pa to M. It is then clear
that, (p^ipp = 4>a for every a < /3. Let TV be an iZ-module and for every
© 2003 by CRC Press LLC
a ? S. let. tpa : N —> Ma be iZ-homomorphisms such that, (p^ijjp = tpa for
every a < /3. For y ? N. the element (ipa(y)) of T\Ma is in M. Define
9 : N —> M by 9(y) = (ipa(y))., y ? N. The map 9 is an iZ-homomorphism.
4>a6 = ipa and 9 is the only homomorphism with this property.
We thus have
Theorem 1.6.19 The module M with homomorphisms 4>a : M —»¦ Ma, for
every a ? S, is the inverse limit of the inverse system
Remark 1.6.20 Although direct and inverse limits can be defined more
generally by using categorical / functorial language, we have preferred to
give only the classical approach.
Remark 1.6.21 Let {Ga,n^}s be a direct family of groups over a directed
set S. Let X = \Jaes^a 'De *^e disjoint union of the groups Ga. Tf
x, y G X, then there exist a. P G S such that x G Ga. y G G13. Say that
x ~ y if there exists a 7 > a, ft such that n^x = TtZy. The relation ~ is
an equivalence relation on X and let. G denote the set of all equivalence
classes into which X is partitioned. Let [x] denote the equivalence class
determined by x G X. Let x G Ga. y G C3 and choose 7 > a,/?. Define
[x][y] = [^axnjy]- The product defined is independent of the choice of the
representatives x in [x] and y in [y] and G becomes a group. For a € S.
let na : Ga -»¦ G be the map defined by 7ra(a;) = [x], x G Ga. Each ?ra
is a group homomorphism. The group G together with homomorphisms
na. a ? Sis called the direct limit of the directed family {Ga,n^}s
of groups. As in the case of modules it. can be proved that, direct, limit,
of a directed family of groups is unique upto isomorphism. Also it can be
proved that every group is direct limit of the family of its finitely generated
subgroups directed by inclusion.
1.6.22 Exercises
The results listed in the following exercise are very much a part of the
theory but we are omitting the details.
1. If S is a directed set and {Ma,n^}s is a direct, family of right
iZ-modules. {Na,nP}s is a direct family of left iZ-modules. M is a right
iZ-module and /V is a left iZ-module. prove that
(a) {lim^Ma} ®RN^ lim^{Ma ®R N, ?rf ® 1} ;
(b) M ®R Um^{Na,nP} ^ lim^{M ®R Na, 1 <g> 71^}.
2. Let {Aa, tt^} and {Ba, 0^} be direct, families of i?-modules over the
same directed set S. By a homomorphism A : {Aa,na} -»¦ {Ba,(f)P} we
mean a family of iZ-homomorphisms Aa : Aa —> Ba such that for every
Let {Aa,nP}: {Ba,^a} and {Ca,tpP} be direct, families of ^-modules
over the same directed set. 5 and A : {Aa, tt%} -> {Ba, </>%}. ji : {Ba, </%} ->
© 2003 by CRC Press LLC
{Ca,i[)P} be homomorphisms of direct families such that, for every a ? S.
0 -> Aa h Ba ^ Ca -> 0
is an exact sequence. Show that there is an exact sequence
0 -> \im{Aa,nP} A \im{Ba,4>P} A lim{CQ,^} -> 0.
3. Let {Aa,TT^} be a direct family of left iZ-modules over a directed set
S and R be any left iZ-module. Show that
(a) HomR(\lm^{Aa,^},B)=\lm^{HomR(Aa,B^
(b) HomR(B, lim^{Aa, tt^}) S*]im^{HomR(B, Aa),
1.7 Pull Back and Push Out
Although pull back of a diagram and push out of a diagram can be defined
in any Abelian category, we here define these and study their existence and
uniqueness only for iZ-modules.
Definition 1.7.1 An iZ-module P together with R-homomorphisms / :
P —> A and q : P —> B is called a pull back of the pair of R-homomorphisms
a : A -»¦ C and 0 : B -»¦ C if (i) the diagram
commutes ; and (ii) if X is another R-module with a pair of homomorphisms
h : X -»¦ A, j : X -»¦ R such that the diagram
X
commutes ; then there exists a unique R-homomorphism 9 : X —> P such
that. fO = h, and gO = j.
© 2003 by CRC Press LLC
Proposition 1.7.2 Every pair of R-homomorphisms a : A
B —> C has a pull back.
C and
Proof. Take P = {(a,b) G A © B\a(a) = 0{b)} and define / : P -> A
and g : P —> B by /(a, 6) = a. </(a, 6) = b. (a, b) ? P i.e. /. g are just the
natural projections : A © _B —> A and : A © _B —>¦ B restricted to P. The
maps / and g are iZ-homomorphisms and af = fig.
Let X be an iZ-module with homomorphisms h : X ->¦ A, j : X ->¦ B
such that ah = [ij. Define 6» : X -> P by 9{x) = (h(x),j(x)). x G X.
Since a(/i(x)) = fi(j(x)), (h(x),j(x)) does indeed belong to P. The map #
is an _R-homomorphism such that. fO(x) = h(x) and g6(x) = j(x) for every
x G X. Therefore f6 = h and g6 = j. Hence (P. f, g) is a pull back
diagram of the pair a : A —)¦ C. /?:/?—>¦ C. q
Proposition 1.7.3 // (P. /. g) and (P'; /'. g') are iwo pwH feacfe o/
the homomorphisms a : A —»¦ C. /3 : B —)¦ C, then there exists a unique
R-isomorphism 0 : P —»¦ P' .smc/i tftrrf ttfi diagram
commutes.
Proof. Since (P'; /'. g') is pull back of the pair (a. /3) and the diagram
P
commutes, there exists a unique iZ-homomorphism 0 : P —>¦ P' such that
/'^ = /. g'^ = g. Also (P. /. g) being a pull back of (a. /?). there exists a
unique iZ-homomorphism <f> : P' —>¦ P such that f4>=f- g<p = <?''• Then,
we get
and
Also /lp = /. #lp = g and /'lp< = /'. .g'lp' = .g'. Uniqueness of the map
in the definition of pull back then shows that <p6 = lp and 9(f> = \p<. Hence
© 2003 by CRC Press LLC
9 is an isomorphism with cf> as its inverse. Since we have already proved the
commutativity of the diagrams
B
the proof is complete, q
Definition 1.7.4 Given a pair of i?-homomorphisms a : A —»¦ B. C : A —>¦
C. a module P together with module homomorphisms / : B —> P. g :
C —> P is called a push out of the pair a, ft if fa = gj3 and if X is
another module with homomorphisms /' : B —> X. g' : C —> X such that
fa = g'P, then there exists a unique homomorphism <f> : P —> X such that
<pf = /' and <f>g = g'. Tn terms of diagrams we say that P together with
/. g is a push out if the diagram
commutes and if X is another module with homomorphisms /' : B
g' : C -»¦ X such that the diagram
X.
commutes, then there exists a unique homomorphism <f> : P —> X such that,
the diagrams
© 2003 by CRC Press LLC
B
B
and
X
— X
commute.
Proposition 1.7.5 Every pair of homomorphisms a : A
C has a push out.
B and 13 : A
Proof. Take P = B®C/K where K is the submodule K = \(a(a), -/3(a))\
a G A} of B e C. Define / : B -> P and g : C -> P by /(&) =
F,0) + K, b G 7? and g(c) = @,c) + if. c G G. The maps /. g are
clearly iZ-homomorphisms and for any a G A
(fa)(a) = f{a{a)) = {a{a),0) + K
Therefore fa = g/3.
Let X be another ii-module and /' : B -> X. g' : C -> X be i?-
homomorphisms such that fa = g'0. The map <f> : B © G ->¦ X given
by <j)((b,c)) = f'(b) + g'(c), b ? B. c G C is an i2-homomorphism and it
vanishes on K. Therefore it induces a homomorphism <p : P —> X. (j)((b, c) +
K) = f'(b) + g'(c) and <pf = /'. <f>g = g'. Let ip : P -> X be another R-
homomorphism such that, ipf = /'. ^^ = g'. For b ? B. c G C.
= fib) + g'{c) = 0(F, c)
so that if> = <f>. q
Proposition 1.7.6 If P with f : B -> P, g : C -> P and P' with f :
B —»¦ P'. q1 : C —>¦ P' are two push outs of the pair of homomorphisms
a : A —»¦ B. C : A —»¦ C, then there exists a unique isomorphism <p : P —> P'
such that 6q = a': d>f = f. i.e. the diaqram
© 2003 by CRC Press LLC
is commutative.
Proof. Exercise.
1.7.7 Exercises
1. Prove Proposition 7.6.
2. Tf 7T : Z/12Z -> Z/3Z and tt' : Z/15Z -> ZjiZ are the natural
projection maps and P with / : P ->¦ Z/12Z, g : P ->¦ Z/15Z is the pull
back of the pair (tt, tt'). prove that P is a cyclic group of order 60 and /. </
are again the natural projections.
3. Find push out of the diagram
Z/12Z-
a
Z/6Z
P
Z/4Z
Z.
where a. /3 are the homomorphisms induced by the identity map : 2
Is the push out P of this diagram cyclic ? What is its order ?
4. Find conditions on integers m. n and t so that, in the push out of
the diagram
Z/mZ-
a
Z/nZ
z/tz
the Z-module P is cyclic.
5. Find conditions on integers m. n and t so that in the pull back of
the diagram
Z/tZ
Z/mZ-
a
P
Z/nZ
the Z-module P is cyclic.
6. Consider a push out diagram
© 2003 by CRC Press LLC
of the pair a. /?. Prove that, if a is a monomorphism. so is the homomor-
phism g. If a is an epimorphism. is g an epimorphism ?
7. Consider a pull back diagram
of the pair a, /?. Prove that if a is an epimorphism. then so is g. Is the
converse true ? Justify. If a is a monomorphism. is g a monomorphism ?
Justify.
8. Tn the push out diagram of Exercise 6 above, prove that / induces
an isomorphism : Coker a —> Coker g and g induces an isomorphism
: Coker 0 -> Coker /.
9. Tn the pull back diagram of the Exercise 7 above, prove that g
induces an isomorphism : Ker f —> Ker /3 and / induces an isomorphism
: Ker g —> Ker a.
© 2003 by CRC Press LLC
Chapter 2
CATEGORIES AND
FUNCTORS
Tn homological algebra we are concerned with study of derived functors of
additive functors but. for these it. is necessary to recall additive categories.
In this chapter we introduce the concept of categories and functors and
give a number of examples of both. Functorial properties of HoniR(A,B)
and C ®r B are discussed. Also Hom(—, —) and — $S — are proved to be
bifunctors.
2.1 Categories
Definition 2.1.1 By a category C we mean
(a) a collection of objects denoted by objC or simply C:
(b) for every pair of objects A. B in C a set of morphisms denoted by
Mor(A,B) or Hom(A,B):
(c) a law of composition for morphisms i.e. a map :
Mor(A, B) x Mor(B, C) ->¦ Mor(A, G) in which the image of the pair (/, g)
of morphisms where / G Mor(A,B) and g G Mor(B,C) is denoted by gf
and the law of composition satisfies the associative law i.e. if A, B. C. D
are objects in C. f G Mor(A,B),g G Mor(B,C) and h G Mor(C,D) then
h(gf) = (hg)f:
(d) for every object A in C a unique morphism in Mor(A, A) denoted
by 1a called identity morphism of A such that /1a = f f°r every / G
Mor(A,B) and lAf = f for every / G Mor(B,A).
Remark 2.1.2 The sets of morphisms Hom(A,B) for A. B are required
to be mutually disjoint. Tf / G Hom(A,B),g G Hom(C,D), where A, B.
C, D G C , then / cannot equal g unless A = C.B = D. This, in particular,
means that, even if A = C. B is a subset, of D say. in the category of sets
41
© 2003 by CRC Press LLC
and / : A —»¦ B,g : A —>¦ D are maps such that. g(a) = /(a) for all a ? A.
the maps / and g are not equal.
(ii) For every object A ? C. identity element 1a ? Hom(A,A) is unique.
Tf l'A G Hom(A,A) is another identity element then I'^Ia = 1a-, 1'a being
identity and l'A \a = l'A, \a being identity. Hence \a = VA and is as such,
called the identity corresponding to the object A.
To fix our ideas about a category and our notations we consider some
examples.
2.1.3 Examples
1. The category S of sets in which the objects are sets, for sets A.
B, Mor(A,B) is the set of all maps : A ->¦ B: for objects A, B, C and set
maps / : A —»¦ B,g : B —»¦ C, gf denotes the usual composition of maps
and it. satisfies the associative law; for any set A, \a '¦ A —> A is the map
Ia(a) = a for every a ? A. That flA = / for every / G Mor(A,B) and
1af = / for every / G Mor(B,A) is clear.
2. The category Q of all groups in which the objects are groups, for
groups G, G'. Mor(G, G") is the set of all group homomorphisms from G to
G"; for groups G, G', G" and / G Mor(G,G'): g G Mor(G',G"): gf : G ^
G" denotes the usual composition of maps and is a group homomorphism;
for every group G the map 1q '¦ G —> G given by 1g{x) = x. x G G is a
group homomorphism and it. satisfies laf = / for every / G Mor(G' ,G)
and /1g = / for every / G Mor(G,G'). The usual composition of maps
satisfies the associative law and. so. is true for group homomorphisms.
3. The category Ab of Abelian groups in which the objects are
Abelian groups; for Abelian groups G and G'. Mor(G,G') is the set of all
group homomorphisms; the composition of morphisms is the same as in the
category of all groups and. so. satisfies the associative law; 1q '¦ G —> G
is the identity map satisfying the required property IqJ = f for every
/ G Mor{G',G) and flG = f for every / G Mor(G,G').
4. The category rM of (left) iZ-modules in which the objects are
^-modules; for ^-modules A, B, Mor(A,B) = HomR(A,B) the set of all
iZ-homomorphisms : A —> B: and 1a '¦ A —> A for an iZ-module A is the
identity map 1,4(a) = a, a G A.
We can similarly consider the category of all right R-modules which
we denote by Mr.
5. The category TL of all rings in which the objects are rings, for
rings R. S. Mor{R, S) is the set of all ring homomorphisms : R —»¦ S and
lp : R —> R is the usual identity map of sets. it. is a ring homomorphism
satisfying flu = /, lsg = g for f,g ? Mor(R,S). The composition is the
usual composition of homomorphisms.
6. The category Top of all topological spaces, the objects being
topological spaces, for topological spaces X, Y, Mor(X, Y) is the set of con-
© 2003 by CRC Press LLC
tinuous maps: X —> Y: lx '¦ X —> X is the identity map; the composition
of morphisms is the usual composition of maps.
7. Given two complexes or O-sequences
A ¦ -± A drl±1 A 'hi A -±
A .¦•¦->¦ An+l -> An An-\ -»¦••¦
R . _^ R Srl±1 R ^3. R _s.
.d .•••—»¦ nn+i —? tin —t r>n-i —?•••
of i?-modules. by a translation or morphism or a chain map : A —> B_
we mean a family {/„} of B- homomorphisms. /„ : An —>¦ Bn such that
finfn = fn-idn for all n or that the diagram
^ dn+i ^ dn ^ ^ ^
/n.
In
fn-1
•- Bn+i —-—>- Bn —-—>- Bn-i >- ¦ • ¦
<Wl On
is commutative. Consider the family of all complexes of i?-modules and
homomorphisms. For any two complexes A. B_. let Mor(A,M) be the set
of all translations from A to B_. If A. B_. C_ are three complexes and {/„} :
A —> B_. \gn} : B_ —>¦ C_ are chain maps, then gnfn is a homomorphism
: An —> Cn and we get a family of morphisms \gnfn} '¦ A_—* G.- If ?7 is
o_ . • ¦ • —t On_)_i r On —f On —i —t • ¦ •
then for any n. dn(gnfn) = (dngn)fn = {gn-iK)fn = gn-i(Snfn) =
gn-i(fn-idn) = (ffn-ifn-i)dn- Therefore {gnfn} ¦ A -> Q_ is a chain
map. That this composition of chain maps satisfies the associative law
follows from the composition of module homomorphisms satisfying the as-
associative law. Given a complex A we take the chain map 1 = {ln} : A —> A.
where for every n. ln '¦ An —> An is the identity map. For every chain map
{/«} : A -> B_ the compositions {ln}{fn} = {/«} and {/n}{ln} = {/„}.
We thus have a category of complexes denoted by Cornp.
Definition 2.1.4 Let C be a category. A morphism / : A -»¦ B is said
to be an isomorphism or invertible or an equivalence if there exists a
morphism g : B —> A in C such that gf = \a and fg = ls- If g' : B —> A
is another morphism in C such that g' f = \a and fg' = 1#. then
g = Ug = W f)g = g'ifg) = g'iB = g' ¦
Thus g with fg=^B and gf = 1a is uniqualy determined by /. We write
g = f~l and call it the inverse of the isomorphism /. Also observe that,
then g is also invertible with / as its inverse. Hence. (J) = g~l = f ¦
If / : A —> B is an isomorphic morphism in C. then the objects A,B of C
are said to be isomorphic or equivalent objects.
© 2003 by CRC Press LLC
An object 0 in a category C is called a zero object if for every object
A of C the sets Mor(A,O) and Mor(O,A) are singleton sets i.e. each
contains exactly one element and since, lo G Mor(O, 0). Mor(O, O) = lo-
Let 0' be another zero object in C. Then Mor(O,O') and Mor(O',O)
consist, of one element, each. Let / : 0 —> O',g : 0' —> 0 be these unique
morphisms. Then gf ? Mor(O,O) and fg ? Mor(O',O') and. therefore.
gf = lo, fg = lo' ¦ Hence O, O' are equivalent. Thus, zero element of C. if
it exists, is unique upto equivalence.
Every category need not have a zero object. For example, the category
of sets does not have a zero object while the group of order one i.e. the
identity group is a zero object in the category Q as well as in the category
Ab of Abelian groups. The zero module {0} is the zero object in the cate-
category rA4 of i?,-modules and also in the category MR of right i?-modules.
The complex in which every entry is the zero module is the zero object in
the category of comlexes. Since we have restricted our attention to only
rings with identity, the category of rings does not possess the zero object.
However, if we do not insist, on every ring to be a ring with identity, we
can still have a category of rings but this category does have a zero object,
namely the zero ring.
If we do not require both the sets Mor(X,A) and Mor(A,X) to have
one element, ea.ch but. only one of these to have exactly one element, we get
objects in a category which are called initial or terminal objects. Thus,
an object A in a category C is called an initial object of C if for every object
X of C the set Mor(A,X) consists of exactly one element and is called a
terminal object if for every object X of C the set Mor(X,A) consists of
exactly one element. For example, in the category S of sets every singleton
set \a} is a terminal object while such a set is not an initial object. The
empty set <j> is an initial object in S while there can be many terminal
objects. If C is a category with zero 0 (say), then 0 is an initial as well as
a terminal object in C.
Definition 2.1.5 A category C is called a subcategory of a category V
if every object of C is also an object of T> and if A. B are any objects in C.
then Mor(A,B) in C is contained in Mor(A,B) in V.
Tf C is a subcategory of T> and for every pair A. B of objects of C.
Mor(A,B) in C is equal to Mor(A,B) in V. then C is called a full sub-
subcategory of T>.
Obseve that both Q and Ab are subcategories of S but. neither is a full
subcategory while Ab is a full subcategory of Q.
Definition 2.1.6 Let C be a category. A category Cop the objects of which
are the same as those of C is called opposite category of C if for every
pair of objects A. R of C there is one to one correspondence between the
set of morphisms Mor(A,B) in C and the set of morphisms Mor(B,A) in
Cop such that when /. g are morphisms for which gf is defined and /°. g°
© 2003 by CRC Press LLC
are the morphisms in Cop corresponding to / and g respectively, then f°g°
is denned and f°g° = (gf)°.
We can then talk of the opposite category of Cop by taking (/°)° = /.
Thus (Cop)op = C. If 1° denotes the identity morphism corresponding to
the object A in the category Cop then for any / ? Mor(B,A) we have
l^Af = /. Tn particular 1^1A = 1A as well as l^U = 1A. Hence 1A = 1A
i.e. identity morphism corresponding to the object A in C as well as Cop is
the same.
If 0 is the zero object in C. there being one-to-one correspondence be-
between the elements of Mor(A,B) in C and those of Mor(B,A) in Cop. 0
is also the zero object in Cop. Also if A is an initial object in C. then A
is a terminal object in Cop and a terminal object in C is an initial object
in Cop. If / is an isomorphism in C then /° is an isomorphism in Cop and
conversely. The opposite category Cop is also called dual category of C.
Definition 2.1.7 A category C is called preadditive if
(i) C has a zero object;
(ii) for every pair of objects A. B of C the set Hom(A,B) is an Abelian
group w.r.t. addition;
(iii) for all objects A. B. C of C there is a bilinear map Hom(A,B) x
Ham(B, C) ->' Hom(A, C)
i.e. for f,g? Ham(B,C), h,k? Hom(A,B)
(f + g)h = fh + gh; f(h + k) = fh + fk.
For example, the categories Ab. rM. A4r. Cornp are preadditive but. the
catgories <S. Q are not preadditive. The category of rings although has
properties (ii) and (iii) but. does not have the zero object and . as such. it.
is not a preadditive category. However, the category of all rings which are
not necessary rings with identity is a preadditive category. The category
Top of all topological spaces is not preadditive.
2.1.8 We have defined products of a family of i?-modules in two ways
: by considering sequences of elements and then using universal property.
Using the universal property we can introduce the concept of products in
a category.
Definition 2.1.9 Let \Xi},i ? I. be a family of objects of a category C
indexed by a set /. An object X of C with morphisms pi : X —> Xi for every
i ? T called projections is called a product of the family {X^}, i ? T if
for every object Y of C and morphisms fi : Y —> Xi for i ? T there exists
a unique morphism / : Y -»¦ X such that pif = fi for every i ? I i.e. for
every i ? I the diagram
© 2003 by CRC Press LLC
X
fi
is commutative.
We have already proved that, products exist, in the category n-M. of R-
modules. As a special case of this the category Ab of Abelian groups also
has products. These are called direct product of i?-modules or of Abelian
groups. We may also mention that the category Q of groups has products
called direct product of groups, the category S of sets has products in
the form of cartesian products. In each one of the categories mentioned
products are unique upto isomorphism. We now prove that the same holds
in an arbitrary category, if products exist in it.
Theorem 2.1.10 If X with projections pi : X —»¦ Xi, i ? I and X' with
projections p\ : X' —> Xi, i ? I are two products of a family {Xi}. i ? I of
objects in C, then X, X' are isomorphic objects of C.
Proof. Since {X,pi}, i G / is a product in C and p\ : X' -»¦ Xi, i ? I are
morphisms. there exists a unique morphism / : X' —> X such that pif = p\
for every i ? I.
Again {X',p':}. i ? I being a product in C and pi : X —> Xi. i ? I
being morphisms. there exists a unique morphism g : X —> X' such that
Pi9 = Pi f°r every i ? I. Then we have
Pif9 = Pi f°r every i ? I and p[gf = p\ for every i, ? I. Also lx '¦ X —>
X and \x> '¦ X' —> X' are morphisms in C such that Pilx = Pi for every
i ? T and p\\x' = p\ for every i ? T. Using the uniqueness of existence of
morphism in the definition of products we get fg = lx and gf = \x' ¦
Hence / is an isomorphism with g as its inverse. Therefore the objects
X. X' are isomorphic. q
We are now ready to define additive categories.
Definition 2.1.11 A pre-additive category C is called an additive cate-
category if every pair of objects in C has a product.
The categories Q, Ab, nM. Mr, Cornp are additive categories while
the category S is not an additive category.
2.1.12 Every category need not have products. Consider, for example,
the category of all simple groups. This category has the zero object namely
the group consisting of identity alone. If H, K are two simple, non-Abelian
© 2003 by CRC Press LLC
groups then Hom(H, K) cannot be given the structure of an Abelian group.
Thus the category is not pre-additive. Suppose that the category admits
products. Let H. K be simple groups and a simple group X with homo-
morphisms a : X —> H. /3 : X —> K he the product of H and K (re-
(recall that, product is unique upto isomorphism). Consider the homorphism
1h '¦ H —> H and the zero homomorphism : H —> K i.e. the homomor-
phism which maps every element of H onto the identity element. By the
definition of product there exists a unique homomorphism / : H —> X such
that af = 1h,PJ = 0. Then / is a monomorphism and we can regard
H as a subgroup of X. The conditions af = 1H and 0f = 0 then show
that. a\H = \h and 0\H = 0. On the other hand considering the group
K with homomorphism Ik '¦ K —> K and the zero map K —>¦ H we can
regard K as a subgroup of X and that a\K = 0 and j3\K = Ik- That
a\H = 1h shows that a is not the zero homomorphism unless H = {e}
and a | K = 0 shows that. K C Kera C X. Similarly H C Ker/3 C X.
Thus, unless both H and K are trivial groups. X has a non-tririal normal
subgroup which contradicts the simplicity of X. Hence this category does
not admit products.
2.1.13 Exercises
1. Tf / : A —> B and g : B —»¦ A are i?-homomorphisms such that
gf = \a-, prove that / is a monomorphism and g is an epimorphism.
2. Prove that the category Top of topological spaces has a product.
3. Prove that a morphism / : A —> B in the category of sets is an
isomprphism if and only if the map / is both one-one and onto.
4. Construct a category other than the category of simple groups which
does not admit products.
Definition 2.1.14 In an additive category C. a kernel of a morphism
/:/?—>¦ C is defined to be a map i : A —»¦ B such that fi = 0 and that is
universal w.r.t. this property, i.e.. if j : A' —> B is another morphism such
that fj = 0. then there exists a unique morphism p : A' —»¦ A with j = ip.
Diagramatically :
the composition A —> B —> C is zero and if the composition A' —> B —> C
is also zero, then there exists a unique morphism p : A'
diagram
A such that the
A
C
is commutative.
© 2003 by CRC Press LLC
Dually a cokernel of / : B —> C in C is a morphism tt : C —> D such that.
tt/ = 0 while if 7 : G —> D' is another map such that 7/ = 0. then there
exists a unique morphism 7' : D —> D' with 7 = j'lr. Diagramatically :
the composition B —)¦ C —± D is zero and if B —)¦ C A- D' is also zero, then
there exists a unique morphism 7' : D —>¦ D' such that the diagram
B—U
is commutative.
Definition 2.1.15 Tn any category C. a map or morphism a : A —> B
is called a monic if the induced map a* : Hom(C,A) -»¦ Hom(C,B) is
injective for every object C in C. i.e.. if af = af implies / = /' for all
/. /' : C —> A. i.e.. a is left cancellable.
Dually a morphism /3 :/?—>¦ C in any category C is said to be an epic
if the induced map C* : Hom(C,A) —> Hom(B,A) is injective for every
object A in C. Thus /3 epic means f/3 = f'/3 always implies / = /'. i.e.. /3
is right cancellable.
2.1.16 Exercises
1. Tf i : A —> B and j : A' —$¦ B are two kernels of a morphism
/:/?—>¦ C. prove that there is an equivalence between the objects A. A'.
2. Let C be the category nM: Mn or Ab. If i : A ->¦ B is a kernel
of a morphism / : B -»¦ C in C. prove that A = Ker f (Hint. Prove that
j : Kerf —> B with j the inclusion map is a kernel of / : B —> C in the
present sense).
3. Tf 7T : C -»¦ D and (f> : C -> D' are two cokernels of a morphism
/ : i? —>¦ C in C. prove that there is an equivalence between the objects D
and D'. '
4. Let C be the category rM. M.r or Ab. If tt : C —> D is a cokernel
of a morphism / : B —> C in C. show that D = Coker / (= the cokernel of
the homomorphism /). (Hint. Prove that the natural projection p : C —>
Coker f is a cokernel of / : B —> C).
5. In the category tzM- M-tl or Ab. prove that a morphism a : A —»¦ B
is a monic if and only if a is a monomorphism and a morphism ft : B —> C
is an epic if and only if C is an epimorphism.
Definition 2.1.17 An Abelian category is an additive category such
thai
© 2003 by CRC Press LLC
(a) every morphism in C has a kernel and a cokernel;
(b) every monic in C is the kernel of its cokernel;
(c) every epic in C is the cokernel of its kernel.
2.1.18 Some more examples
We close this section by giving examples of a couple of categories which
are quite useful in representation theory. The discussion of path algebras
and minimal morphisms follows closely the doscussion in Auslander. Reiten
and Smalo.
(a) An oriented or directed graph F = (ro,ri) where Fo is the set of
vertices and Fi is the set of arrows between vertices or the set of edges
is called a quiver. We consider here only finite quivers i.e. those
quivers T = (Fo,Fi) in which the sets Fo and Fi are finite. Given a quiver
F = (Fo,Fi) we can define two maps s : Fi —> Fo and e : Fi —> Fo where
s(a) = i and e(a) = j when a : i —> j is an arrow from the vertex i to
the vertex j. A path in the quiver F is an ordered sequence of arrows
p = an ¦ ¦ ¦ aii with e(ai) = s(aii+i) for 1 < i < n or is just a symbol et for
i ? To- The paths e^ are called trivial paths. We define s(ei) = e(ei) = i
and for a non-trivial path p = an ¦ ¦ ¦ ai we define s(p) = s(aii) and e(p) =
e(ain). A non-trivial path p with s(p) = e(p) is called an oriented cycle.
Let k be a field. By a representation (V, /) of a quiver F over the field
k we mean a set of vector spaces {V(i) \ i ? Fo} together with fc-linear
maps fa : V(i) -»¦ V(j) for each arrow a : i ->¦ j. A representation (V, /) of
F is called finite dimensional if for every i ? Fo; the vector space V(i) is
finite dimensional over k. We here consider only finite dimensional
representations.
By a morphism h : (V, /) —> (V',f) between two representations of
the same quiver F over k we mean a collection {hi : V(i) —> V(i)}i^r0 of
fc-linear maps such that, for ea.ch arrow a : i —>¦ j in F the diagram
v'U)
commutes. For two morphisms h : (V, f) —> (V',f) and g : (V',f) —>
(V", /") between representations the composition gh is defined to be the col-
collection of maps {gihi :V(i)—> V"(i)}igr0- The composition of morphisms
trivially satisfies the associative law. Identity morphism corresponding to
a representation (V, /) is the collection {ly(i)}ier0 where ly(i) is the iden-
identity map of the vector space V(i). We thus get the category of finite
dimensional representations of F over k and denote it by RepF.
© 2003 by CRC Press LLC
For two morphisms g. h : (V, /) —> (V',f) between two representa-
representations we define the sum g + h : (V, /) —> (V',f) to be the collection of
maps {gi + hi : V(i) —> V'(i)}i^r0- With respect to this addition the
set Mar((VJ)., (V',f)) of all morphisms : (V, f) -> (V',f) becomes an
Abelian group. Also the map Mor((VJ). (V, f'))xMor((V'J'). (V", f"))
-> Mor((VJ).. {V",f"))&veaby{h,g)^gh,g G Mor((V>J>).. {V",f'%
h G Mor((V,f). (V',f)) is clearly biadditive. By taking O(i) = the zero
space over k for every i G To, we get a representation (O, 0) which is clearly
a zero object in Rep F. Thus the category RepF is a preadditive cate-
category. Since for a representation (V, /) we want all vector spaces V(i) finite
dimensional, the category Rep F is not additive.
Let h : (V, /) —> (V',f) be a morphism between two representations
over the same quiver F. If a : i —> j, /3 : j —> I are arrows in F. then it is
fairly easy to see that hifpfa = faf'ahi- We may thus define fpa = fpfa%
and. in general, for any path p = an ¦ ¦ ¦ a\. we define fp = fan ¦ ¦ ¦ fai and
we have htfp = fpht when s(p) = i and e(p) = t.
(b) We next consider a category which is closely rela.ted to the a.bove
category.
Letfc be a field. F = (Fo,Fi) be a finite quiver and kT the vector space
over k with the paths of F as basis. For paths p. q in F define the product
pq if e(q) = s(p) and p. q are nontrivial.
0 otherwise
Extend this product of paths to the elements of kT by using linearity and
assuming that the elements of k commute with paths in F. Product of paths
in F is associative and. therefore, so is the product in kT. It may then be
checked that kT is an algebra over k and it is called the path algebra of
F over k. For any a = ^ aipt in kT.
°( ^ ej) = 7^ ae3 = J~] J~] aiPiei = J~] aiJ^Piej = Y\ Wi = a..
as s(pi) is some e, so that piej = Pi whereas for other j' in Fo; Piey = 0.
Similarly (^. ej)a = a. Thus X]ier0 e* ^s ^e identity element of kT.
It is clear from the definition of products of paths that for i ^ j in
Fo; e| = ei and e^ej = 0. Therefore {e^ | i G Fo} is a set of mutually
orthogonal idempotents in kT. Recall that an element e of a ring is called an
idempotent if e2 = e. Also, two idempotents e, e' are called orthogonal
if ee' = 0.
If p is an oriented cycle in F. then p2. p3,¦ ¦ ¦ are again paths in F and
are all distinct. Therefore kT is infinite dimensional over k. On the hand
if F has no oriented cycle, the number of paths in F is finite. We thus have
© 2003 by CRC Press LLC
Proposition 2.1.19 If k is a field and T is a finite quiver, then kT is a
finite dimensional k-algebra if and only if F has no oriented cycles.
The following is quite easy.
Proposition 2.1.20 Let V be a quiver with no oriented cycles and J be
the ideal of the path algebra kT generated by all the arrows ofT. Then J is
nilpotent.
We denote by f.d.(fcF) the category of kT-modules of finite k-
dimension.
As for RM and Mr- f.d.(fcF) is a preadditive category and since we
consider only finite fc-dimensional fcF-modules. the category f.d.(fcF) is not.
additive.
(c) For a fixed (left) R-module C, consider the set of all i?-homomorphisms
/ : B -> C. If / : B -> C. f : B' -> C are two iMiomomorphisms. by
a morphism g :/—>/' we mean an i?-homomorphism g : B —> B' such
that the diagram
B
f
C
r
B'
commutes. The identity map 1b ¦ B -»¦ B gives rise to the identity mor-
morphism If which trivially has the property glf = g and I/ft, = h for all
morphisms g :/->/' and h :/'->/. Tf g :/->/'. h : f -> f" are
morphisms. the i?-homomorphisms g : B —> B'. h : B' —> B" induce an
i?-homomorphism hg : B —»¦ B". It is easily checked that the diagram
B
f
C
hg
B"
is commutative. This way we get a morphism hg : f —»¦ /". This composi-
composition of morphisms satisfies the associative law. We have a category which
we denote by Mod R/C and call it the category of morphisms into C.
A morphism g :/—>¦/' is an isomorphism in Mod R/C if there
exists a morphism h :/'—>¦/ such that hg = 1/ and gh = If. This is so
© 2003 by CRC Press LLC
if and only if there exists an i?-homomorphism h : B' —»¦ B such that the
compositions B -4 B' -»¦ B and B' ->¦ B -4 B' are the maps \b and \B>
respectively. But then g :/?—>¦ B' is an isomorphism with ft, : B'—>¦/? as
its inverse. Hence g :/—>/' is an isomorphism if and only if g : B —> B'
is an isomorphism.
A morphism / : B —> C in Mod R/C is called right minimal if
every morphism g : f —> f is an automorphism. This is equivalent to
saying that / is right minimal if and only if every g : B —> B with fg =
f is an automorphism of B. For /. /' G Mod R/C. say that / ~ /'
if Hom(f,f) ^ cj) and Hom(f',f) ^ 4>. The relation '~: is clearly an
equivalence relation and Mod R/C is partitioned into a set of disjoint
equivalence classes.
To settle the existence of right minimal morphisms. we need to recall a
few results from modules.
Definition 2.1.21 An R-module M is called simple if whenever A is a
siibm,odule of M then either A = 0 or A = M. An R-module M which is
direct sum of simple R-modules is called semisimple.
Definition 2.1.22 A (left) R-module A is said to be of finite lenth if
there exists a finite series of submodules A = Aq D A\ D • • • D An = 0 such
that every quotient Ai/Ai+\ is a simple R-module. Such a series is called, a
composition series for A. Jordan-Holder theorem, says that if a module
has a finite composition series, then the number of composition factors in
any composition series is the same. This number of composition factors is
called the length of the module A and is denoted by l(A).
Lemma 2.1.23 Let O^-A-^B^-C^-Obean exact sequence of R-
module.i wth B of finite length. Then the modules A, C are of finite length
andl(B) =
Proof. For simplification of notation we take a to be the inclusion map
and C = B/A. Let B = Bo D B\ D • • • D Bn = 0 be a composition series
for B. Then we have finite series A = Ao D Ai D ¦ ¦ ¦ D An = 0 and
C = Co D Ci D ¦ • ¦ D Cn = 0 for A and C where for any i, 0 < i < n,
Ai = An Bt and Ci = BiA/A(= Bi/(Bi n A)). For any i, we have an exact
sequence
O^Afl Bi/(A n Bi+1) -> Bt/Bl+1 -> Bi/(A n Bi)Bl+1 -> 0
in which the middle term Bi/Bi+\ is a simple module. Therefore, for every
i. either At/Ai+i is simple and Cj/Cj+i = 0 or At/Ai+i = 0 and Cj/Cj+i
is simple. By removing repititions from the series of A and of C. we get
composition series of A and C with number of composition factors for B
equal to the sum of the numbers of composition factors for A and for C.
Therefore, l(B) =l(A) + l(C). n
© 2003 by CRC Press LLC
Proposition 2.1.24 Every equivalence class in Mod R/C containing some
/:/?—>¦ C with B of finite length cantains a right minimal morphism, which
is unique upto isomorphism.
Proof. In the given equivalence class choose / : B —> C with the length
l(B) smallest, possible. Let g : f —> f be a morphism in Mod R/C. Let
f\g(B) = f¦ We then have a commutative diagram
g{B)
- B
If g(B) ± B: then l(g(B)) < l(B) and / : g(B) -> C is a morphism in
Mod R/C. This contradicts the choice of /. Hence g(B) = R and g is an
epimorphism.
Let K = Kerg. Then K C Kerf and / induces a morphism / :
B/K -> C. If K ^ 0. the exa,ot. sequence 0 -> if -> B -> 5/if -> 0 implies
that Z(-B) = l(K)+l(B/K) > l(B/K) which again is a contradiction. Hence
if = 0 and g is an isomorphism. Therefore / : B —> C is right minimal.
Let /' : B' —> C be a right minimal morphism which is equivalent to
f : R -> C. There then exist morphisms g '¦ f -> f g' : /' ->¦ /• Thus
//'g and gg' are morphisms : / —> f and : /' —> /' respectively. Since
both / and /' are right minimal morphisms. g'g and gg' are isomorphisms.
Hence g and g' are isomorphisms. Therefore right minimal morphism in an
equivalence class is unique upto isomorphism, rj
For a fixed i?-module A, we may consider the category Modi?—A whose
objects are i?-homomorphisms / : A -»¦ R. If / : A -»¦ R and f':A->B'
are two morphisms. a morphism g :/—>/' is an i?-homomorphism <? :
B ^ B' such that the diagram
7?'
commutes. As a dual of the notion of right minimal morphism. we call
a morphism / : A —»¦ B left minimal if whenever g : B —> B is an R-
homomorphism such that, gf = f, then g is an automorphism. We may
© 2003 by CRC Press LLC
define an equivalence relation in the category Modi? — A exactly the way
it is done in Modi?/C. Corresponding to Proposition 1.24 we have the
following for left minimal morphisms.
Proposition 2.1.25 In every equivalence class in Mod R — A of a mor-
phism f : A —»¦ Y with Y of finite length, there exists a left minimal mor-
phism which is unique upto isomorphism.
2.1.26 Exercises
1. The category RM or Mr or Ab is an Abelian category.
2. Give an example of a category which is additive but is not Abelian.
3. Give an example of a category which is pre-additive but is not addi-
additive.
In homoloqical algebra we shall, in general, be concerned with category
of modules.
2.2 Functors
Definition 2.2.1 Let C and T> be two categories. We say that. F is a
covariant functor from the category C to the category T> and express it
by F : C -> V if
(i) F attaches a unique object F(A) of V to every object A of C:
(ii) F attaches to a morphism / : A —> B in C a unique morphism
F(f) : F(A) -> F{B) in V such that, if / : A -> B, g : B -> C are
morphisms in C, then F(gf) = F(g)F(f).
(iii) FAa) = ^-f(A) for every object A in C.
Definition 2.2.2 Let C and T> be two categories. We say that. F is a
contravariant functor from the category C to the category T> and express
it. by F : C -> V if
(i) to each object A of C is associated a unique object F(A) of T>\
(ii) to each morphism / : A —> B in C is associated a unique morphism
F(f) : F(B) -> F(A) in the category V such that, if / G Mor(A,B),
g G Mar(B, C) in C then F(gf) = F(f)F(g).
(iii) FAa) = 1f(tI) f°r every object A in C.
If C and ?> are pre-additive categories . a functor F : C —»¦ ?> whether
covariant or contravariant is called additive if F(f + g) = F(f) + F(g) for
every /, g G M or (A, B) in C.
2.2.3 Examples
To fix our ideas about functors we consider some examples.
1. For any group G define T(G) = G/G', where G' = [G,G] is the
derived group of G. Then T(G) is an object in Ab. If G. 7? are groups
© 2003 by CRC Press LLC
and / : G —> H is a homomorphism, then / induces a homomorphism
T(f) : G/G' -> H/H' which is defined by T(f)(aG') = f(a)H', a ? G. Tf
f : G —)¦ H. g : H ^ K are homomorphisms of groups, it is clear from
the definition of induced homomorphisms that T(gf) = T(g)T(f). That
T{1q) = Iq/G' f°r every group G is also clear. Thus, we have a functor
2. For any nonempty set X. let. T(X) denote the free group (or free
Abelian group or free R-module with X as a basis). Then any map / :
X —> Y. where X. Y are sets induces a homomorphism on the free objects
T(f) : T(X) -> T(Y) and we get a functor T : S -> Q (or Ab or ^JW).
Since the categories ?/ and <S are not preadditive. the functors defined
in the above two examples are not additive.
3. Let X be an i?-module. For every i?-module A define T(A) =
Ho7tir(X, A) which is an Abelian group. If A, B are i?-modules and / :
A —> R is an _R-homomorphism define T(/) = i?om(l, /) : HoniR(X, A) —>
HomR(X,B) by
T{f){a) = fa, a?HomR(X,A).
The map T(f) is clearly a homomorphism. Let A, B. C be i?-modules
and / : A —»¦ B. g : R —> C be i?-homomorphisms. Then gf : A —> C is an
R-homomorphism and for every a ? Hottir(X, A).
T(gf)(a) = (gf)a = g(fa) = T(g)(fa) = T(g)(T(f)(a)) = (T(g)T(f))(a).
Therefore T(gf) = T(g)T(f). That TAA) = 1T{A) for any i?-module A is
clear. Hence T = HoniR(X, —) is a covariant. functor :r M. —> Ab.
Again, if A, B are i?-modules and f,g? Hottir(A,B). then f + g ?
Hottir(A,B) and for any a ? Hottir(X,A).
T(f + g)(a) = (f + g)a = fa + ga = T(f)(a)+T(g)(a) = (T(f)+T(g))(a).
Therefore T(f + g) = Hom(l,f + g) = Hom(l,f) + Hom(l,g). Hence
T = HoniR{X, —) is an additive covariant. functor.
Instead of considering left i?-modules, we could consider right i?-modules
in the above and we, similarly, get an additive covariant functor Hoitir(X, -)
: Mr -»¦ Ab . where X is a right i?-module.
4. Again let X be a left .R-module and for any left i?-module A we get
an Abelian group T(A) = Hottir(A,X). Tf A. R are i?-modules and / :
A —> B is an i?-homomorphism define T(f) = Hom(f, 1) : Hottir(B, X) —>
HomR(A,X) by
T(f)(a) = af, a?HomR(B,X).
T(f) is clearly a homomorphism of Abelian groups and T = Hoitir(-,X) :
rM. —> Ab is a contravaria,nt additive functor.
© 2003 by CRC Press LLC
By replacing left i?-modules by right i?-modules in the above we shall
get a contravariant functor
T = HamR(-,X) :MR^Ab
If R is a commutative ring then for i?-modules A, B. Hottir(A,B) is
again an i?-module. Therefore the functors defined in examples 3 and 4 are
functors from the category of i?,-modules to the category of i?-modules.
5. Let M be a fixed right i?-module and TV a fixed left i?-module. Then
Ti :r M -»¦ Ab and T2 : Mr ->¦ Ab defined by
T^A) =M®rA: AeRM
T2(B) =B®rN,B?Mr
are covariant. additive functors. In case R is a commutative ring then
M®rA and B®rN are i?-modules and. as such, both T1; T2 are functors
from the category of i?-modules to the category of i?-modules.
6. For an Abelian group M and positive integer n. let SPn(M) denote
the nth symmetric power of M i.e. SPn(M) = M §5 • ¦ -®MjI. where
/ is the subgroup of M®n = M <3 ¦ ¦ ¦ ® M generated by all elements of the
form x-\ ® x2 §5 • • • ® xn - Xn(i) ® x^^) (8 • • • ® ^(n): where !;? M and
ir ? Sn the symmetric group of degree n defined on the set {1,2, • • • ,n}.
Then T : Ab ^ Ab defined by T(M) = SPn(M),M G ^4&; is a covariant.
functor.
7. Let G be a group (written multiplicatively). R, be a commutative
ring and let i?.f? denote the group ring of G over R. Let /(??, i?) denote the
augmentation ideal of RG i.e. I(G,R) = \Y.riXi G RG\ E^i = 0} =
the ideal of i?G generated by all elements of the form x — 1, a; G G. In fact
/(??, i?) is also a free i?-module with {x - l\x G G, x ^ e} as its basis. For a
positive integer n, let In(G, R) denote the nth associative power of I(G, R).
Let Pn>R(G) = I(G,R)/In+l(G,R) and Qn,R{G) = In(G,R)/In+l(G,R).
Then Pn,R(G) and Qn,R(G) are i?-modules. Define T : Q ^ M {= Mr =
RM) and 5 : Q -> 7W by
T(G) = PntR(G): S(G) = Qn>R(G), G?0.
Then T. 1.9 are covariant functors from the category of groups to the cate-
category of i?-modules.
We write I(G,R) = I(G), Pn,R{G) = pn(G) and Qn<R(G) = Qn(G)
when R, is the ring Z of integers.
8. Let R be a commutative ring. For a group G. let T(G) = RG. Then
T is a covariant functor from the category of groups to the category of
rings.
9. Consider the category Comp of complexes of i?-modules. Let n be a
natural number (or even an integer). For a complex
A :•¦¦->¦ Am+1 d^ Am H Am_i
© 2003 by CRC Press LLC
let Hn(A) = Kerdn/Imdn+l which is an i?-module. If A. B are two
complexes of i?-modules and f = {fn} : A —>¦ B is a chain map. then fm
restricted to Ker dm takes values in Ker d'm and fm restricted to Im dm+i
takes values in Imd'm+l. Thus f induces homomorphisms /^ : Hm{A) —>¦
Hm(H) which are defined by
H(f)(a + Imdm+i) = /„(«) + Imd'm+1, a G Kerdm.
Then Hn is a covariant additive functor : Comp —>r M.
2.2.4 Exercise
Prove that the functors Pn : Q ->¦ Ab, Qn:Q^tAb and SPn : Ab -»¦ Ab
as defined in examples 6 and 7 above are non-additive functors.
Definition 2.2.5 Let C and V be two categories and T, U : C -> V be
two functors either both covariant. or both contravariant. We say that.
li : T —> U is a natural transformation (of functors) if for every object
A in C there is associated a morphism ha : T(A) —> U(A) in T> such that
for every morphism / : A —> B in C. the diagram
T(A)
T(f)
T(B)
U(A)
U(f)
U(B)
if both T and U are covariant or the diagram
T(f)
T(B) —-*
T(A)
U(B)
U(f)
U(A)
if both T and U are contravariant. is commutative.
Tf for each A in C. ha '¦ T(A) -»¦ U(A) is an equivalence, then /j, is a
called a natural equivalence and we write it. as /i : T % U. Also then T
and U are called naturally equivalent functors.
© 2003 by CRC Press LLC
2.2.6 Examples
1. Let n > 1 be given. When G is an Abelian group, then 8n(G) :
SPn(G) -> Qn{G) denned by
9n(G)(Xl ®x2---®xn) = {xx- l)(x2 - 1) ¦ • ¦ (xn - 1) + In+l(G):
Xi ? G. is a well defined homomorphism (infact an epimorphism). Tf H is
another Abelian group and / : G —> H is a homomorphism. then we have
a commutative diagram
Spn{G) ^?L Qn(G)
SPn(f)
US)
SPn{H) . Qn(H)
0n(H)
We thus have a natural transformation 9n : SPn -»¦ Qn.
2. For any Abelian group G. 02(G) : SP2(G) -»¦ Q2(G) is an isomor-
isomorphism (cf. Passi [32]. Theorem VIII 8.6). Thus 02 : <SP2 -> Q2 is a natural
equivalence. However 8n : SPn —> Qn for n > 2 is not a natural equiv-
equivalence. For this. let. G = Zp ffi Zp direct, sum of two cylic groups each of
prime order p. Then ( Passi A976), Theorem 4.7) Qn(G) ^ Zpl+p) for all
n > p.
For Abelian groups A B, SPn(A®B) = ® Ei+j=n-5^i(^)«)'5-pi(B);
where it. is understood that. SP°(A) ^ Z. For a cyclic group A, S'Pn(A) =
A for all n > 1. Therefore, for the group G considered above. SPn(G) =
Zpn+l). Hence for n > p, SPn(G) is not isomorphic to Qn(G) showing
that 0n : SPn ->¦ Qn is not a natural equivalence for n > 2 because for any
such n. we can choose a prime p with n > p and then take G = Zp © Zp.
3. Let C denote the category of torsion free Abelian groups. Tf G is
a torsion free Abelian group, then so is SPn(G) (cf. [47]) and 6n(G) :
SPn(G) -»¦ Qn(G) is an isomorphism. Hence 0n '¦ SPn -»¦ Qn is an equiva-
equivalence for all n for the functors SPn : C -> C and Qn : C -> C.
Let ? be another ring with identity.
Definition 2.2.7 A functor T : rM —> sM{orMs) is called an exact
functor if whenever A1 —)¦ A —> A" is an exact sequence in rM. then
the sequence T(A') TH] T(A) TH] T(A") is exact, if T is covariant and
the sequence T{A") TH] T(A) TH] T(A') is exact, if the functor T is
contravariant.
© 2003 by CRC Press LLC
Definition 2.2.8 A functor T : RM -> sM(orMs) is railed left exct
if whenever 0 -»¦ A' ->¦ A -4- A" ->¦ 0 is an exact sequence in ^jVf. then the
sequence 0 -> T(A') T^) T(A) T^) T(A") is exact, if T is covariant while
the sequence 0 -> T(A") T^) T(A) T^) T(A') is exact, if T is contravariant.
Definition 2.2.9 A functor T : RM -»¦ sJW(otMs) is called right ex-
exact if whenever 0 —> A' —> A A A" —> 0 is an exact sequence in rM. .
then the sequence T(A') TH} T(A) TH] T{A") -> 0 is exact, if T is covari-
covariant while the sequence T(A") TH] T(A) TH] T(A') -> 0 is exact, if T is
contravariant.
Lemma 2.2.10 Let A -»¦ R -4 C and C A- D -»¦ K 6e exacf sequences of
R-modules and homomorphisms. Then
(i) the squence A —»¦ /? -^ D is exact, if i is a monomorphism.
and
(ii) the sequence B -^ D —>¦ -B «s exact, if g is an epimorphism.
Proof. Exercise.
Proposition 2.2.11 A covariant functor T : rM —> s-M-(orAis) is left
exact if and only if 0 —> A' —)¦ A —> A" is an exact sequence in rM. implies
that 0 -> T(A') TH] T(A) TH] T{A") is an exact sequence.
Proof. Exercise.
Proposition 2.2.12 A contravariant functor T : rM. —> Ms *s left exact
if and only if whenever A' —> A —> A" —> 0 is an exact sequence in R, then
the sequence 0 -> T(A") TH] T(A) TH] T(A') is exact.
Proof : Let A' -»¦ A -4 A" ->¦ 0 be an exact sequence in RM. Let
K = Ker f.L = Ira /(= Ker g) . Then we have exact sequences
O-y^^i'lf^O, O-yf,4i4i"->O
where i, j are inclusion maps and / = jf.
If T is left exact, we get exact sequences
0 -> T(L) TH] T(A') TM] T(K)
and
0 -> T(A") T^) T(A) ^ T(L).
© 2003 by CRC Press LLC
Lemma 2.10 then shows that, the sequence
0 _> T(A") TH] T(A) T(/4T(i) T(A')
is exact. Since T(f)T(i) = T(if) = T(f), T is left exact.
The converse is trivial, rj
Proceeding on the same lines we can also prove
Proposition 2.2.13 For covariant functor T : rM. —> sM{orMs) the
following are equivalent :
(a) T is right exact;
(b) Whenever A' —)¦ A A A" —)¦ 0 is an exact sequence in rM, then
the sequence T(A') TH] T(A) TH] T{A") -> 0 is exact.
Proposition 2.2.14 For contravariant functor T : rM —> s-M- the fol-
following are equivalent :
(a) T is right exact;
(b) Whenever 0 —> A' —)¦ A —> A" is an exact sequence in rA4, then
the sequence T(A") TH] T(A) TH] T(A') -> 0 is exact.
2.2.15 Examples of equivalent categories.
Definition 2.2.16 Let C and T> be two categories and, F : C —»¦ T> and,
H :T> -> C be functors. If the functors HF : C -> C and FH : V -> V
are naturally equivalent to the identity functors 1 : C —»¦ C and 1 : T> —> T>
respectively, the categories C and T> are said to be equivalent.
Our aim here is to prove that the categories Rep V and f.d. kT for a
given finite quiver V over a field k are equivalent categories.
Let F = (Fo, Fi) be a finite quiver over a field k.
(a) For (V, f) in the category Rep F of finite dimensional representations,
let. F(V,f) = ®EieraV(i). For each i G F0; let. m : F(V,f) -> V(i)
be the natural projection and A; : V(i) —> F(V,f) be the natural injection
associated with the direct sum decomposition so that ttjAj = ^v(i), ttjA^ =0
for i ^ j and 5Zier •^7r« = ^F(v,f)- By definition of a representation, for
each arrow a : i —> j in Fi we have a fc-linear map fa : V(i) —> V(j).
More generally, for a path p in F there is a fc-linear map fp : V(i) —> V(t)
where s(p) = i and e(p) = t. There is then induced a fc-linear map fp =
^tfpKi '¦ F(V,f) —> F(V,f). For the trivial path e^ the induced map is
fe. = Aj/e,.7Tj. where fei : V(i) —> V(i) is the map ly(i)- Extend the map
fp to the path algebra kT by linearity. We thus get a fc-linear map / : kT —>
Endk(F(V, /)). which is indeed a fc-algebra morphism (this follows from the
© 2003 by CRC Press LLC
definition of fp in the last, section). Using the fc-algebra morphism /. we
can make F(V, /) into a fcF-module. Explicitly for a G kT. v G F(V, /), we
define av = fa(v). The map / being an algebra homomorphism. F(V,f)
does indeed become a fcF-module.
Let h : (V,f) -»¦ (V',f) be a morphism in Rep F. Then we have a
fc-linear map hi : V(i) —> V'(i) for every i ? Fo and hence an induced map
h : F(V, /) -> F(V',f) of vector spaces : h(vi) = (hi(vi)), vt G V(i).
For each path p with s(p) = i, e(p) = t in F we have htfp = fpht.
Therefore, for v = (vi, ¦•¦,«,,•••) ? F(V, /) with Vi ? V{i).
hfP(v) = h\tfpTTi(v) = h\tfp(vi)
= h@, • • •, fp(vi),0, • • •) = @, • • •, htfp(vi),O, • • •)
¦)= fph(v)
which proves that. hfp = fph. This then implies that. hfa = f'ah for every
it G kT. But then h(av) = ah(v) for a G fcF. v G F(y, /). showing that /?, is
a fcF-map. We define F(h) = h. To prove that F is a functor we need only
to check that (i) h — 1_f(v,/) if hi = ^v(i) for every i and (ii) h'h = h'h for
morphisms h : (V, f) -> (V', /') and /i' : (V', /') -> (V", /")¦ But these are
clear from the definition of the morphism h.
(b) Consider an object C in f.d.(fcF) i.e. C is a finite dimensional kT-
algebra. Tf order of Fo is n. we have 1 = e\ + • • • + en which is sum of
orthogonal idempotents in kT and we can write C = ffiJ^eiC. For each
it G kT. we can define a map /„¦ : C —> C by fa{c) = ac. c G C. If a : i —> j
is an arrow in F we have
aifiiC) = (aei)C = aC = e3aC C e?C;
as aC C C. Therefore a induces by restriction to e^C a fc-lineax map
fa : tiC —> ejC. We define H(C) to be the representation (V,f). where
V = {eiC | i G Fo} and for each arrow a : i —> j in F. fa is as defined
above.
If B. C are two elements in f.d.(fcF) and h : B —> C is a morphism in
this category, we have h{eiB) = eih(B) C aC. Therefore h restricted to
eiB gives a fc-linear map hi : eiB —> tiC. Since h is a fcF-homomorphism.
for an arrow a : i —> j in F and b G B. ah(b) = ha(b) which implies that
ahi(b) = hj(ab) for b G eiB. We get H(h) = {hi}. For an arrow a : i -»¦ j,
it. is clear from the definition of hi and /a's that, the diagram
© 2003 by CRC Press LLC
e,B
e,C
ejB
ejC
is commutative. Infact we have already proved this above when we said
ahi(b) = hj(ab) for every b G e{B. That HAB) = {le)B} and H(h'h) =
H(h')H(h) for fcF-homomorphisms h : B -»¦ C, h' : C ->¦ D are clear.
Thus we get a functor H : f.d.(fcr) -> Rep T.
Theorem 2.2.17 The categories f.d.(fcF) and Rep T are equivalent.
Proof. For an element. (V, /) in the category Rep T, F(V, /) = ® E V(i)
and let HF(VJ) = (V,g) (say). Then
V(i) = eiF(V,f) = fe,(F(VJ)) = \ifeM
Also for «:?'—>¦ j in F. ga : V(i) —> V(j) is defined by just multiplication
by a i.e.
9a(V(i)) = 9a(eiF(V,f)) = aeiF(y,f)=aF(V,f)
= U(F(VJ)) = Aj/«7ri(F(y,/)) = Xjfa(y(i)).
This proves that for every i ? Fo and a : i —> j in F. the diagram
commutes. The maps A^ being injections (monomorphisms). the horizontal
maps in the above diagram are isomorphism. Hence {A^} : (V, f) —> (V,g)
is an isomorphism of representations. Tn order to prove that the functor
HF is equivalent to the identity functor : Rep F —> Rep F. we only need
to prove that, if h : (V, f) —> (V',f) is a morphism in Rep F. then the
diagram
(VJ)
(V,g)
HF(h)
© 2003 by CRC Press LLC
is commutative. But this is clear from the definitions of F(h) and then
H(F(h)). Observe that F(h) = (hi) i.e. (h1,h2,---) and H(F(h)) is just
the map F(h) restricted to the iih component for every i G Tq i.e. it is hi
on \i(V(i)).
On the other hand, if C G f.d.(kT), then H(C) = (V,f), V(i) = efi.,
and for a : i —> j in F. fa : V(i) —>¦ V(j) is just multiplication by a. Now
F(V, f) = 8 E V(i) =8 E eiC = C and for a : i -> j in F;
= Aj/Q(eiC) = Aj(aeiC) = Aj(aC) = aC.
Thus identifying C with © E ejC under the isomorphism c —»¦ (ejc). c ? C.
we find that FH(C) = C. If B. C are finite dimensional fcF-algebras and
/ : B —> C is a fcF-morphism. the diagram
/
B C
where in the lower horizontal map the map e,i? —> eiC is just the restriction
of / to eiB. is commutative, q
2.2.18 Exercises
1. Is the category Rep T preadditive. additive or Abelian ?
2. Is the category f.d.(fcF) preadditive. additive or Abelian ?
3. If the categories Rep F and f.d.(fcF) are preadditive. are the functors
F and H additive ?
2.3 The Functors Horn and Tensor
In this section we study exactness properties of the two Horn functors and
the two functors obtained through tensor product. We then define bifunc-
tors and show as examples that. Homu(—, —) and — <2)r — are bifunctors.
Theorem 2.3.1 Let X be a left R-module. Then both Homu(X,—) and
Homn(—,X) are left exact functors.
Proof. Consider an exact sequence 0->A->B4Gof left _R-modules
and _R-homomorphisms. Applying the functor Homu(X, —) to this se-
sequence we get a sequence.
B.1) 0 -> HomR(X, A) 4 HomR(X, B) 4 HomR(X, C)
© 2003 by CRC Press LLC
where /*. g* are defined by f*(a) = fa. a G HomR(X,A): g*(a) = ga.
a G HomR{X,B).
Suppose a G HomR(X, A) satisfies f*(a) = 0. Then fa = 0 and. so for
any x G X. 0 = fa{x) = f(a(x)) which implies. / being a monomorphism.
that. a(x) = 0. Hence a = 0 and /* is a monomorphism.
For any a G HomR(X, A).
<?*(/») = <?*(/") = «?(/«) = («?/)« = 0,
as Ira f = Ker g.
Therefore Im f* C Ker g*.
Finally let f3 G HomR(X, B) such that g*(/3) = 0. Then gf3 = 0 and for
a; G X. 0 = (gP)(x) = g(/3(x)). Thus /3(z) G ATer g = Im a and. so. there
exists a unique a ? A (unique because / is a monomorphism) such that
/3(x) = f(a). Define a : X —> A by a(x) = a. where a G A is the unique
element, with j3{x) = f(a).
Let x,y G X. r G R. Suppose a,a' G A such that. 0(x) = f(a) and
/%) = f{a'). Then
a(a;) = a: a(y) = a'.
Now. as P and / are i?-homomorphisms.
/3(rx) = r/3(x) = rf(a) = f(ra)
and
P(x + y)= p(x) + p(y) = f(a) + f(a') = f(a + a').
Therefore
a(rx) = ra = ra(x)
and
a(x + y) = a + a' = a(x) + a(y).
Hence a is an i?-homomorphism. Also it. is cleax from the definition of
a that. /3 = fa = f*(a). Therefore Ker g* C Im f* and. so. Im f* =
Ker g*. This completes the proof that the sequence B.1) is exact and.
hence. Homn(X, —) is left exact.
In order to prove that Homn(—,X) is left exact we have to prove that if
A —> R A- C —>0isan exact sequence of left i?-modules then the sequence
0 -> HomR{C,X) 4 HomR{B,X) 4 HomR{A,X) is exact. We leave the
details to the reader, q
Remark 2.3.2 Neither of the two functors HomR(X, -) and HomR(-,X)
is exact. For this, first observe that Hom(A,B) = 0 if A is any torsion
Abelian group and B is a torsion free Abelian group. Let m be an integer
> 2. Then we have an exact sequence
© 2003 by CRC Press LLC
B.2) 0->Z™Z4 Z/mZ -> 0
where m : Z —> Z is the multiplication map by m. Applying the functor
Hom(Z/mZ, —) to this sequence, we get an exact sequence
0 -> Hom(Z/mZ, Z) -> Hom(Z/mZ, Z) ^ Hom(Z/mZ, Z/mZ)
Now Hom(Z/mZ,Z) = 0 whereas 1 : Z/toZ -> Z/toZ given by l(a +
mZ) = a + raZ. a G Z. is a non-zero homomorphism showing that
Hom(Z/mZ, Z/mZ) ^ 0. Hence n* is not. an epimorphism and the functor
Hom(Z/mZ, —) is not exact.
Again, applying the functor Hom(—, Z/mZ) to the exact sequence B.2).
we get an exact sequence
0 -> Hom(Z/mZ, Z/mZ) ^ Hom(Z, Z/mZ) % Hom(Z, Z/mZ).
For any / G Hom(Z, Z/mZ), and a G Z.
m*(f)(a) = (fm)(a) = f(ma) = mf{a) = 0.,
as /(a) G Z/mZ which is of order m. Hence m* : Hom(Z, Z/mZ) —>¦
Hom(Z, Z/mZ) is the zero map whereas Hom(Z, Z/mZ) = Z/mZ ^ 0.
Therefore m* is not an epimorphism and. so. Hom(—, Z/mZ) is not an
exact functor.
Regarding the functor Homu(X, —) we can have the following.
Theorem 2.3.3 If F is a free left R-module, then Homji(F, —) is an exact
functor.
Proof Consider an exact sequence 0 —> A —> B A C —> 0 of R-
modules and homomorphisms. To prove the result we only need to show
that, g* : Homu(F,B) —> Homu(F,C) is an epimorphism. Let F be free
with basis X and a G Homu(F,C). For any x G X, a(x) G C. Choose
b G B such that. a(x) = g(b). Define a map fj : X -> B by j3(x) = b,
where b G B is the element chosen to satisfy g(b) = a(x). The module F
being free on X. extend the map /3 to a homomorphism /3 : F —»¦ B. Since
gC{x) = a(x) for every x G X. g/3 = a on F i.e. g*(/3) = a. Thus g* is an
epimorphism and the functor Hompi(F, —) is exact. [—]
Theorem 2.3.4 If X is a right R-module, then the functor X ®r — :
rM —> Ab is right exact.
Proof. Consider an exact sequence
B.3) A 4 B 4 C -> 0
© 2003 by CRC Press LLC
of left R-modules. Applying the functor X ®p — to this sequence, we get
a sequence.
B.4) X ®R A ^V X ®R B l%9 X ®R C -> 0
of Abelian groups. Here 1 = lx is the identity map : X —> X. For any
x G X, a ? A
A <g> g)(l 0 f)(x <8) a) = A g) fl)(a; (8) /(a)) = a; (g) c?(/(a)) = 0,
as g(f(a)) = 0 . the sequence B.3) being exact. This proves that Ira A $5
f)cKer(l®g).
Let M = Im A <g> /) and consider the quotient group X ®R B/M. Since
M C Ker (l(g)p); l®g induces a homomorphism g : X®RB/M -^ X®RG.
where 5B; ® 6 + M) = x ® pF). a; G X. fteB.
Define a map h : X x C —> X ®R B/M as follows :
If x G X. c G C. choose b ? R such that g(b) = c and set
h(x,c) = x 0b + M.
If ft' G -B is another element, such that. g(b') = c. then g(b' — b) = 0 and
there exists an a G A such that b' — b — f(a). Then
This proves that, the map h is well defined. Extend the map h to a ho-
momorphism h from the free Abelian group Z(X, C) with basis X x C
to X ®fl B/M. Let a;. Xi, a;2 G X, c, cu c2 G C. r G i? and
choose 6; 61. 62 G R such that pF) = c. <?(&«) = Cj. z = 1,2. Then
= re and pFj + 62) = Ci + C2. Therefore
/i(a;7-, c) - /i(a;, re) = xr <g> 6 - a; ® rft + M = 0;
h{x, ci + c2) - h(x, ci) - h(x, c2) = x (g> (bi + b2) - x (g> bi - x (g> b2 + M = 0
h(xi +X2,c) - h(xi, c) - h(x2,c) = (xi + x2) §§ b - Xi ® 6 - x2 ® 6 + M = 0.
It. therefore, follows from the definition of tensor product that, h induces a
homomorphism h: X®RC ^ X®RB/M, such that. /i(a;®c) = x®b + M.
where b ? R such that g(b) = c.
Then
3/1B; (g) c) = 7?(a; ® 6 + M) = x ® pF) = 2; (g) c
showing that 5/1 = identity map on X ®R C.
Also, trivially, for x G X, ft G -B,
/iff(a; ® ft + M) = h(x ® #(&)) = a; ® ft + M
showing that Kg = identity map onl® B/M.
Thus ~g is an isomorphism with h as its inverse.
© 2003 by CRC Press LLC
Let -k : X(g>RB ->¦ X®RB/M be the natural projection. Then gir = l0g
and ~g being a monomorphism
Ker A ® g) = Ker (~gn) = Ker n = M = Ira A ® /).
Finally, consider x G X. c G C. Let b G B such that c = g(b). Then
a;(8>c = x®g(b) = (l®g)(x®b) which proves that 1® </ is an epimorphism.
This completes the proof that the sequence B.4) is exact. Hence X &R —
is right exact. [—j
Remark 2.3.5 The functor X ®r — is not exact. For this we first need
to prove that, if D is a divisible Abelian group and A a torsion Abelian
group, then D®A = A®D = 0. Let a G A and x G D. Let order of
a be n. Since D is divisible, there exists y G D such that, x = ny. Then
x $5 a = (ny) $5 a = y $5 (no) = y $5 0 = 0. Similarly a <g> x = 0. Hence
Let to be an integer > 2. Tensoring the exact sequence.
B.5) 0-yZ4Q4 Q/Z -> 0
where Q is the additive group of rational numbers, with ZjraZ over Z. we
get an exact sequence
ZjmZ ® Z ^ ZjmZ ® Q ^ Z/mZ ® Q/Z -> 0.
Since Q is a divisible group and Z/raZ is a finite group. Z/raZ <g> Q = 0.
But ZjraZ & Z = Z/raZ ^ 0. Therefore. 1 <g> i is not a monomorphism.
Thus Z/raZ $5 — is not an exact functor.
Theorem 2.3.6 If X is a left R-module, then the functor —®rX : M.r —>¦
Ab is right exact.
Proof. Exercise.
The functor — <g>fl X is not exact. The functor — $5 ZjraZ applied to
the exact sequence B.5) shows that.
is not a monomorphism.
We need the following simple result at several places.
Proposition 2.3.7 Let
B.6) 0^i4fi4C->0
© 2003 by CRC Press LLC
be a split exact sequence of R-modules and homomorphisms, M be a left
R-module and N a right R-module. Then the sequences
B.7) 0 -> HomR(M, A) 4 HomR(M, B) 4 HomR(M, C) -> 0;
B.8) 0 -> HomR{C, M) 4 HomR(B, M) 4 HomR(A, M) -> 0
exact.
B.9)
are again
Proof. Since the functors HomR(M, —) and HomR(—, M) are left exact,
for the exactness of B.7) and B.8) we need only to prove that, g* and /*
in B.7) and B.8) respectively, are epimorphisms. Also the functor N <8>r —
being right exact, for the exactness of the sequence B.9) we need only prove
that 1 (g> / is a monomorphism.
Since the sequence B.6) splits, there exist i?-homomorphisms h : B —»¦ A
and k : C —»¦ B such that, hf = \a and gk = la-
Let h* : HomR(A,M) —> HomR(B,M) be the homomorphism induced
by h. Then, for any a G HomR(A,M).
(f*h*)(a) = f*(h*(a)) = f*(ah) = (ah)f = a{hf) = alA = a
which implies that. f*h* = identity map. This proves that, not only /* is
an epimorphism, but that, the sequence B.8) is split exact.
If k* : HomR(M, C) -»¦ HomR(M, B) is the homomorphism induced by
the homomorphism k : C —> B. we can prove that g*k* = identity map
which then implies that the sequence B.7) is split exact.
Observe that A ® /i)(l ® /) = 1 ® (hf) = 1 $5 1a = identity map and
then not only 1 $5 / is a monomorphism but the sequence B.9) splits as
well. [-]
Definition 2.3.8 Let A, B. C be categories. We say that. T : A x B -> C
is a bifunctor covariant in both variables if the following hold :
(a) For every object A in A and for every object B in B. T(A, -) : B -»¦ C
and T(—,B) : A —»¦ C are covariant functors:
(b) If / : A —> A' is a morphism in A and g :/?—>¦ B' is a morphism in B
then the diagram
T(A,B)
T(f,lB)
T(A',B)
T(U,g)
T(U',g)
T(A,B')
T(f,lB)
T(A',B')
© 2003 by CRC Press LLC
is commutative.
Definition 2.3.9 Let A, B. C be categories. We say that T : A x B -> C
is a bifunctor contravariant in the first variable and covariant in
the second variable if
(a) for every object A in A., T(A, —) : B —> C is a covariant functor, for
every object B in B. T(—,B) : A —> C is a contravariant functor:
(b) for every morphism / : A' —)¦ A in A and a morphism g : B —> B'
in B the diagram
T(A,B)
T(f,
T(A,B')
T(f,
T(A',B')
is commutative
We may define a bifunctor T : A x B -»¦ C contravariant in both the
variables or covariant in the first variable but contravariant in the
second in a similax fashion.
2.3.10 Examples
1. We have seen earlier that for every left _R-module A. Homn(A, —) :
rM. —> Ab is a covariant functor while Homn(—,A) : rM. —> Ab is a
contravariant functor. Let / : A' —»¦ A and </ :/?—>¦ _??' be homomorphisms.
For any a G Homu(A,B).
= Hom(lA',g)(af)
= g(af)lA' =g(af)
and
= Hom(f,lB')(ga)
= (ga).f = g(a.f)
This proves that, the diagram
© 2003 by CRC Press LLC
HomR(A,B)
Hom(f, 1)
HamR(A',B)
Hom(l,g)
Hom(l,g)
HomR(A,B')
Hom{f,
HomR(A',B')
is commutative.
Hence Horn : RM. x RM. —> Ab is a bifunctor contravariant in the first
variable and covariant in the second variable.
2. For every right -ff-module A and for every left -ff-module B. A ®r
— : RM —> Ab and — ®r B : AiR —> Ab are covariant functors. Let
/ : A —> A' be an i?-homomorphism of right -ff-modules and g : B —> B' be
a homomorphism of left i?-modules. Then
= f®g
and
which proves, that the diagram
B
1 (gig
is commutative. This proves that
- $5 - : A4fl x RM ->¦ ^46
is a bifunctor covariant in both variables.
2.3.11 Exercises
1. If X is a left _R-module. prove that, the functor HomR(—,X) is left
exact and the functor — ®fl X is right exact.
2. Tf F1 is a free -ff-module. is the functor HomR(—, F) exact ? Justify.
3. If D is a divisible Abelian group, prove that the functor Hom(—,D) :
Ab —$¦ Ab is exact.
© 2003 by CRC Press LLC
4. If G ^ {0} is an Abelian group, prove that. Hom(G,Q/Z) ^ {0}.
where Q is the additive group of rational numbers.
5. If to > 2 is an integer, prove that Hom(Z/mZ, Z/mZ) = Z/mZ and
also Z/mZ ® Z/mZ ^ Z/mZ.
6. If to. n are integers both > 2. prove that. raZ + nZ/nZ = dZ/nZ,
where d = g.c.d(m, n). Use it. to prove that. Z/mZ <g> Z/nZ = Z/rfZ.
7. Let to. n. rf be as in Exercise 6 above. Prove that Hom(Z/mZ.
Z/nZ) = kZ/nZ where k = n/d.
8. If D is a divisible Abelian group and A is any Abelian group, prove
that Hom(D, A) is torsion free. If D is. in addition, torison free, then
Hom(D, A) is divisible.
9. If A and B are finite Abelian groups of coprime orders, prove that.
Hom(A, B) = 0 and A ® B = 0.
10. If 0 -»¦ A ->/?->¦ G -»¦ 0 is a split exact sequence of right i?-modules
and M is a left i?- module, then Q^A®rM^B®rM^C®rM^Q
is again split exact.
11. Prove that, the sequence B.7) is split exact.
12. Tf a : A -»¦ B and f3 : B ^ A are -R-homomorphisms such that
/Jet = 1a: prove that a is a monomorphism and /3 is an epimorphism.
13. Let {Ma. n}i be a direct system of Abelian groups over a directed
set /. Prove that, (a) \SPn(Ma), SPn(ii)}I is also a directed system of
Abelian groups and
(b) SPn(lim{Ma, it} ^ lim{5P"(M"); SPn(n)}.
14. If {Ma, ?rf}/ is a directed family of torsion-free Abelian groups,
prove that. lim_> \Ma, n}i is again torsion-free Abelian.
15. If G is a torsion-free Abelian group, prove that. SPn(G) for n > 1
is also torsion-free Abelian.
16. Does the functor Qn, where Qn(G) = In(G)/7"+I (G) for a group
G. commute with direct limit ?
© 2003 by CRC Press LLC
Chapter 3
Projective and Injective
Modules
One of our main aims is to study derived functors of additive functors and.
in particular, the derived functors of the 'Horn' and 'Tensor' functors. For
studying derived functors we need what are called projective and injective
modules. Tn this chapter we study some basic properties of these modules.
Among other results, we prove the projective basis theorem and Baer's
criterion for injective modules. It is proved that, every module can be
embedded in an injective module.
3.1 Projective Modules
Definition 3.1.1 A left -ff-module P is called projective if given a dia-
diagram.
P
f
a
of R-modules and homomorphisms with row exact, there exists an R-
homomorphism g : P —> A which makes the completed diagram commuta-
commutative i.e. ag = f.
3.1.2 Two questions arise. Does there always exist a projective i?-module
? If yes. do there exist sufficiently many projective modules ? The second
question is equivalent to saying : Is every module homomorphic image of
a projective module ? We have already answered these questions in the
affirmative. As a consequence of Theorem 1.2.9. we have
73
© 2003 by CRC Press LLC
Proposition 3.1.3 Every free R-module F is a projective R-module.
The second question then answered by the above and Theorem 1.2.8.
3.1.4 Example
We show that, every submodule of a projective module need not be
projective.
Since B. regarded as an i?-module is free. B. is a projective i?-module.
Let p be a prime number and consider R = Zjp2Z. The multiplication
map p* : Z/p2Z -> Z/p2Z, p*(a+p2Z) = pa+p2Z, a G Z, is a Z/p2Z-
homomorphism and Imp* = pZjp2Z.
Consider the diagram
pZ/p2Z
identity map
Z/p2Z
C.1) p*
in which the row is exact. If there is a homomorphism g : pZ/p2Z —> Z/p2Z
which makes the completed diagram C.1) commutative, then for any k ? Z.
we have
pk+p2Z = (p*g)(pk+p2Z) = p*(g(pk+p2Z)) = pg(pk+p2Z) = 0:
which is a contradiction. This proves that the submodule pZ/p2Z of the
projective Z/p2Z-module Z/p2Z is not projective. Thus every submod-
submodule of a projective module need not be projective. The above example
also demonstrates that, every quotient of a projective module need not be
projective.
The following simple observation is extremely useful in comparing dif-
different projective resolutions of modules which we shall study later.
Proposition 3.1.5 Let P be a projective R-module. If in the diagram
P
a C
of R-modules and homomorphisms the row is exact and j3f = 0, then there
exists a homomorphism g : P —> A such that ag = f.
© 2003 by CRC Press LLC
Proof. Let B = Im a(= Ker /3) and a : A —> B be the homomorphism
induced by a. That 0f = 0 implies that image of / is contained in the
Ker /3 = Im a = R. Therefore / induces a homomorphism / : P —> R
such that if i : R —^ R is the inclusion map. then a = ia and / = if. We
have then a diagram
A .- B 0
a
in which the row is exact. The module P being protective, there exists a
homomorphism g : P —»¦ A such that ~ag = f. But then ag = (ia)g =
i(ag) = if = f. Q
There do exist, protective modules which are not free.
Let {Pi}i?j be a family of i?-modules and let. P = 8 ^2j€J Pj-
Then, for every j G J. there exist i?-homomorphisms ij : Pj -»¦ P and
¦Kj : P ->¦ Pj such that
1 if j = k
0 ifi#*
Theorem 3.1.6 The module P is projective if and only if Pj is projective
for every i ? J.
Proof. Suppose that every Pj. j G J. is projective. Consider a diagram
P
f
A >¦ B
a.
with row exact. For j G J. we have a homomorphism / ij : Pj —»¦ B and
the module Pj being projective there exists a homomorphism gj : Pj —»¦ A
such that agj = f ij . Define g : P —> A by
(Observe that, the sum on the right hand side is finite). Then, g is a
homomorphism. For x G P.
© 2003 by CRC Press LLC
which shows that, ag = f. Hence P is projective.
Conversely, suppose that P is projective. For any j ? J. consider a
diagram
Pi
a
with row exact. Then fnj is a homomorphism : P —> B and P being
projective. there exists a homomorphism g : P —»¦ A such that ag = fnj.
Take gj = gij. Then agij = fnjij = f. Thus Pj is projective. q
We now show that, every projective module need not be free.
3.1.7 Example
Let p. q be distinct, primes and consider R = ZjpqZ. The sequence
C.2) 0 -> pZ/pqZ A Z/pqZ ^ qZ/pqZ -> 0
where i is the inclusion map and q* is multiplication by q. is an exact
sequence of i?-modules. The two extreme groups in C.2) being finite of
coprime orders, the sequence C.2) splits. Therefore. pZ/pqZ and qZ/pqZ
are projective i?-modules. If either pZ/pqZ or qZ/pqZ were free. it. will be
direct sum of copies of Z/pqZ. But pZ/pqZ is of order q where as Z/pqZ
is of order pq. Hence neither pZ/pqZ nor qZ/pqZ is free Z/pqZ-module.
Projective modules can be characterized through Horn functor as in
Proposition 3.1.8 An R-module P is projective if and only if the functor
Homn{P, —) is exact.
Proof Suppose that. Homn(P, —) is an exact functor. Consider a dia-
diagram
P
a
B
© 2003 by CRC Press LLC
of modules and homomorphisms in which the row is exact. If C denotes
the kernel of /. applying the functor HomR(P, —) to the exact sequence
we get an exact sequence
0 -> HomR(P, C) lA HomR(P, A) 4 HomR(P, B) -> 0
The homomorphism /* being an epimorphism. there exists a /3 ? HomR(P.
A) such that a = /*(/?) = f/3 which shows that P is projective.
Conversely, suppose that P is a projective .R-module. Since the functor
HomR(M, -) is left exact for every i?-module M, we only need to prove that
HomR{P, —) preserves epimorphisms. Let / : A —> B be an epimorphism
of i?.-modules. For any a ? HomR(P, B). we can find (the module P being
projective) a /3 ? HomR(P, A) such that a = f/3 = /*(/?) which shows that
/* : HomR(P,A) —> HomR(P,B) is an epimorphism. Hence HomR(P, —)
is an exact functor, q
Since every i?-module is a homomorphic image of a free i?-module and
every free i?-module is projective. we have
Theorem 3.1.9 Every R-module is a homomorphic image of a projective
R-module.
Theorem 3.1.10 An R-module P is projective if and only if every exact
sequence 0—> A —> R —> P —> 0 splits.
Proof. Suppose that. P is projective. Consider an exact sequence
C.3) 0->44b4P->0
By definition of a projective module, there exists an i?-homomorphism h :
P —> B such that, gh = lp which shows that, the sequence C.3) splits.
Conversely, suppose that, every exact sequence of the form C.3) splits.
Every module being homomorphic image of a free i?-module. let. F be a
free i?-module and a : F —> P be an epimorphism. If A denotes the kernel
of a. we get an exact sequence 0—> A —> F —> P —> 0 which, by hypothesis,
splits. Thus F = P © A. The i?-module F being free is projective and then
Theorem 1.6 shows that. P is projective. rj
Let P be a projective i?-module and F be a free i?-module with a : F —>
P an epimorphism. If A is the kernel of a, then 0->i->F4P->0is
an exact sequence which splits (Theorem 1.10). Thus.
Proposition 3.1.11 Every projective R-module is a direct summand of a
free R-module.
© 2003 by CRC Press LLC
Theorem 3.1.12 If P is a projective right R-module, then. P ®R — is an
exact functor from RM. to Ab. Similarly, if P' is a projective left R-module,
then — <&RP' is an exact functor from the category M.R to the category Ab.
Proof. Since the functor M®R — is right exact for every right i?-module
M, it is enough to prove that P ®R A -»¦ P ®R B is a monomorphism if
/ : A —> B is a monomorphism of i?-modules. We first prove this for a
free right R-module F in place of P. Suppose that. F = 8 J2ieI Ri: where
Ri = R for every i ? I. Since tensor product commutes with direct, sums.
F ®R A =
A =
the isomorphism being given by (r$) $5 a —> (r* $5 a), where n ? Ri and
a ? A. Also, for every i ? I. Ri (gip A = Aiz where Ai = A. and this
isomorphism is given by rt ® a —> r^a. ri ? Ri. a ? A. Combining the two
isomorphisms we get an isomorphism
:F®RA
iGI
where
The diagram
0{{n) (g>a) = (na)., in) ? F., a?A.
C.4)
F®RB
i)
in which for every i. f\ : Ai —>¦ Bi is the homomorphism / : A —> B, is
commutative. Tn the diagram C.4) the two 0's are isomorphisms as above
and every fi being a monomorphism. (/j) is a monomorphism. Therefore
1 ® / is also a monomorphism.
Since every module is a homomorphic image of a free i?-module. let. F
be a free right i?-module and <f> : F —»¦ P be an epimorphism. The module
P being projective. there exists a monomorphism if> : P —»¦ F such that
4>il> = lp. Then we have homomorphisms
: F
P
: P t
F ®r A
such that.
Therefore ?/> $5 1 is a monomorphism. In the commutative diagram
© 2003 by CRC Press LLC
1®/
the two vertical maps i])®l and the lower map 1 ® / are monomorphisms.
Therefore the upper map 1®/ : P®rA —> P®rB is also a monomorphism.
The proof for the exactness of the functor — (g>/j P' is similar, q
Using the exactness property of the functor A ®r — or — (g)/j B. we can
define
Definition 3.1.13 A right i?-module A is flat if the functor A ®r — is
exact. Similarly a left i?-module B is called flat if the functor — &R B is
exact.
The above theorem shows that every projective module is flat.
Let m be a positive integer. Tensoring the exact sequence 0 —> Z —>
Z -»¦ Z/mZ -»¦ 0 with a flat Z-module A over Z, we find that the multi-
multiplication map m : A —> A is a monomorphism. This implies that every flat
Z-module is torsion-free.
The additive group Q of rational numbers is a flat Z-module (we shall
see this later) but. it. is not a projective Z-module for otherwise it will be a
free Z-module.
We close this section with an extremely useful characterization of pro-
projective modules.
Theorem 3.1.14 (Projective Basis Theorem) An R-module A is pro-
projective if and only if there exists a subset {ai\i ? 1} of A and a set {<fii :
A —> R\i ? 1} of R-homomorphisms such that
(i) for any a ? A, <f>i(a) = 0 for almost all i ? I,
(ii) for any a ? A, a = Y*iei (t>i{a)ai-
Also then A is generated by the elements {a, | i ? /}.
Proof First; suppose that. A is a projective i?-module. Let F be a free
i?-module with a basis {xi\i ? 1} and <f> : F -»¦ A be an epimorphism. The
module A being projective. there exists a homomorphism if> : A —»¦ F such
that <fn]) = 1a-
For any i ? I, define <f>t : A -»¦ R by <j>i{a) = r{, where %j}(a) = J2ieI nxi.
Ti ? R. (pi are well defined; the module F being free on \xi\i ? 1} and
© 2003 by CRC Press LLC
are clearly i?-homomorphisms. Since ip(a) is a finite sum of the form
Y^rixi-, ^i(a) = 0 for almost all i. For i ? I, define a* = <f>(xi). If a € A.
then
a =
Thus (i) and (ii) are proved.
Conversely, suppose that, there is a subset {ai\i ? 1} of A and a set
{<f>i : A —> R\i ? 1} of i?-homomorphisms such that, the properties (i) and
(ii) as in the statement are true. Consider a set X = \xi\i ? 1} of symbols
indexed by the same set / and let F be a free i?-module with basis X. The
map 4>: X —> A given by <j)(xi) = Oj. i ? I, extends to an i?-homomorphism
(j> : F -> A. Ifa?A, then
a = ^2^i(a)ai = ^2^i(a)^(xi) = (f>(^2(t>i(a)xi)
i i i
which shows that, (ft is an epimorphism. On the other hand, define a map
%(> : A -> F by
C.5) i;(a) = J2Ma)xi.
i
Tn view of the condition (i) satisfied by fa, the right hand side in C.5) is a
finite sum. That ij) is an i?-homomorphism follows easily. Also, for a ? A.
i
(by condition (ii)).
Therefore (pip = \a which shows that, the epimorphism <f> splits. Hence A
is a direct, summand of the free module F and is. therefore, projective. rj
3.1.15 Exercises
1. If R = Z/12Z, prove that 3Z/12Z is a projective -ft-module but
6Z/12Z is not projective.
2. Prove that every projective Z-module is a free Z-module.
3. Let R be a principal ideal domain. Assuming that every submodule
of free i?-module is free, prove that every projective i?-module is free. (We
shall come back to this when we study Dedekind domains).
4. Prove that, direct, product of projective modules need not be projec-
projective.
3.2 Injective Modules
Definition 3.2.1 An i?-module E is said to be injective if given a dia-
diagram
© 2003 by CRC Press LLC
A _2_ B
E
of R-modules and homomorphisms with row exact. there exists a homo-
homomorphism g : B —$¦ E which makes the completed diagram commutative
i.e. ga = f.
For looking at some meaningful examples of injective modules, we need
to wait for a while.
Proposition 3.2.2 If E is an injective R-module, then given a diagram
f
E
with row exact and fa = 0, there exists a homomorphism g : C —> E such
that the completed diagram is commutative i.e. g/3 = f ¦
Proof Let X = kernel of C ( = image of a ). Then C induces a
monomorphism ]3 : B/X -> C. ]3(b + X) = /?(&). b G R. Also fa = 0
implies that, image of a is contained in the kernel of / and. therefore. /
induces a homomorphism / : B/X -> E, J(b + X) = f{b), b G B. Let
ir : B —> B/X denote the natural projection. Then f = fir and C = j3n.
Since E is injective. there exists a homomorphism g : C -»¦ E such that
/ = gj3. Then
g/3 = g(/3ir) = (gC)ir = fir = fu
Theorem 3.2.3 Let {Ej}j€j be a family of R-modules and E = Hj€jEj
( = direct product of Ej). Then E is injective if and only if every Ej is
injective.
Proof. Since E = Hj^iEj. there exist, homomorphisms ij : Ej —»¦ E and
Pj : E —»¦ Ej such that, pjij = lEr. anfi Pkij = 0 . the zero map if j ^ k.
Let a : A —> B be a monomorphism of i?-modules.
Suppose that every Ej is an injective i?-module. Let / : A —> E be an
i?-homomorphism. Then pj f : A —»¦ Ej is a homomorphism and Ej being
injective. there exists a homomorphism gj :/?—>¦ Ej such that gja = pjf.
Define g : B -»¦ E by
g(h) = (gj(b)). be B.
© 2003 by CRC Press LLC
Then g is an i?-homomorphism and for any a ? A.
g(a(a)) = (gja(a)) = (pjf(a)) = f{a)
which implies that, ga = f. Hence E is an injective i?-module.
Conversely, suppose that. E is an injective i?-module. For any j G /;
let. fj : A —> Ej be an i?-homomorphism. The module E being injective.
there exists a homomorphism g : B —)¦ E such that ga = ijfj- Then pjg is
a homomorphism : B —»¦ Ej such that
Pjga=pjijfj = fj.
Therefore, every Ej is an injective i?-module. rj
Since direct product of a finite family of i?-modules is the same as the
direct sum of this family of modules, we have
Corollary 3.2.4 Every direct summand of an injective R-module is injec-
injective.
Proposition 3.2.5 An R-module M is injective if and only if the functor
Homn(—,M) is exact-
Proof Since Homn(—,M) is a contravariant left exact functor, it is
enough to prove that. M is injective if and only if whenever 0 —> A A B is
exact then Homn(B,M) %¦ Homn(A,M) ->¦ 0 is exact.
Let an exact sequence 0 —> A ¦% B be given. If M is an injective module.
for every / G Homn(A, M). there exists a homomorphism g : B —»¦ M such
that, f = ga = a* (g) which shows that, a* is an epimorphism. Conversely.
suppose that a* is an epimorphism. Therefore, for every / : A -»¦ M. there
exists a g G Homn(B, M) such that / = a*(g) = ga which shows that M
is injective. rj
Proposition 3.2.6 // E is an injective R-module, then every exact se-
sequence
C.6) O^E^AAB^O
splits.
Proof Exercise.
The converse of the above result, is also true. For the converse we first
need to prove that every module can be embedded in an injective mod-
module. This, unlike every module being a quotient of a projective module, is
much harder to prove. Before we go to proving this result. we consider a
characterization of injective modules given by R. Baer. The proof of this
characterization uses transfinite induction.
© 2003 by CRC Press LLC
3.3 Baer's Criterion
Any proof of a result that uses transfinite induction invariably uses Zorn's
lemma - which is not a lemma needing proof as the name suggests but is
an axiom. We need a version of Zorn's lemma through partially ordered
sets.
Definition 3.3.1 A non-empty set S on which is defined a binary relation
'<' satisfying
(i) x < x for every x ? S.
(ii) x < y and y < x imply x = y. x,y ? S.
(iii) x <y and y < z for x, y, z ? S. imply x < z
i.e. the relation is reflexive, anti-symmetric and transitive, is called a par-
partially ordered set.
3.3.2 Let 5 be a partially ordered set and A be a subset of 5. An element.
x G 5 is called a least upper bound of A if a < x for every a G A and if
a < y for every a ? A. then x < y. Least upper bound of a subset A may
or may not exist but if it exists, it is necessarily unique.
Let N denote the set of all natural numbers and on the cartesian product
N x N define relations ' <' by
r \ i m ^ f x ., 2o+l 2a; + l
(a) (a, b) < (x, y) if —^— < -^—-.
(&) (a. b) < (x. y) if either a < x or a = x and b < y:
(c) (a. 6) < (x. y) if a < x and b < y.
All the three relations are partial order relations but for none of these
relations the partially ordered set N x N has an upper bound and so no
least, upper bound exists.
An element, x G 5 is said to be a maximal element of 5 if a; is not < y
for any y ? S except x. A partially ordered set may have more than one
maximal elements. For example, let S be the set of all proper subgroups
of the additive Abelian group Z of integers partially ordered by inclusion.
For every prime number p. subgroup pZ of Z is a maximal element of S.
Definition 3.3.3 A partially ordered set 5 is called a chain or a simply
ordered set or a linearly ordered set if for every pair of elements x,y
in S either x < y or y < x.
3.3.4 Zorn's Lemma. Let 5 be a partially ordered set in which every
chain has a least, upper bound. Then 5 has a maximal element.
As a beautiful application of Zorn's lemma we have the following cri-
criterion (known as Ba,er's Criterion) for an i?-module to be injective. This
criterion is needed at many places.
© 2003 by CRC Press LLC
Theorem 3.3.5 A left R-module M is injective if and only if for any left
ideal J of R every R-homomorphism : J —»¦ M can be extended to an R-
homomorphism : R. —> M.
Proof. Suppose that. M is an injective left -R-module. Let / be a left
ideal of R. and / : J —> M be an -R-homomorphism. It is immediate
from the definition of an injective module that / can be extended to an
-R-homomorphism : B, —> M.
Conversely suppose that for any left ideal I of R every _R-homomorp-
hism : J —> M can be extended to an -R-homomorphism : R —> M. Let
A, B be left -R-modules. i :/?—>¦ A a monomorphism of left -R-modules
and /:/?—>¦ M be an -R-homomorphism. For the sake of convenience of
notation, we may regard B as a submodule of A and i as the inclusion map.
Let S be the set of all ordered pairs (Bj, fj) where Bj is a submodule of A
with Be Sj and fj : Bj —> M is an -R-homomorphism which extends / i.e.
fj restricted to B is /. For ordered pairs (Bj,fj), (Bk, fk) G S. say that
(Sj, fj)< (B/., fk) if Bj is a submodule of Bk and fk is an extension of /,-.
This relation makes 5 into a partially ordered set. Let {(-Bj, /j)|j G A} be
a chain in 5. Then B' = UBj is a submodule of A. If b G B' . then b G Bj
for some j G A and set f'(b) = /jF).Then /' is a map : B' -> M. Let
b, b' G B' and r, s G B. There exists a k G A such that b, b' G Bk. Therefore
f'(rb + sb') = fk(rb + sb') = rfk(b) + sfk(b') = rf'(b) + sf'(b')
showing that /' is an -R-homomorphism. Clearly /' extends fj for every
j G A. Hence (B',f) is an upper bound of the chain. It is fairly easy
to see that. (B',f) is the least, upper bound of the chain. Zorn's lemma
is thus applicable and 5 has a maximal element. (C,g) (say). We claim
that C = A. Tf C ^ A, there exists an element a ? A, a not in C. Then
C" = C + B.a is a left -R-module such that C c C" C A. Let T = {r G
R\ra G C}. The subset. / is a left ideal of R. Define a map h : I -> M
by h(r) = g(ra). r ? I. The map h is an -R-homomorphism and. so. there
exists an -R-homomorphism a : R —»¦ M such that. a(r) = h(r) for every
r G I. Define g' : C -> M by g'(c + ra) = g(c) + a(r). c G C, r G R. The
map g' is indeed an i?-homomorphism and extends g. Thus (C, g) < (C',g')
and (C,g) ^ (C",g'). This contradicts the choice of (C,g). Hence C = A
and g is an -R-homomorphism : A —> M which extends /. This completes
the proof that M is an injective left -R-module. rj
Corollary 3.3.6 // a left ideal I of R is an injective R-module, then I is
a direct aummand of R.
Definition 3.3.7 An -R-module A is said to be a divisible -R-module if
for every a ? A. and r ? R. r not a zero divisor in R. there exists an
element, b G A such that, a — rb.
© 2003 by CRC Press LLC
Observe that, if R is an integral domain then no r ? R, r / 0 is a zero
divisor in R and A is divisible if for every a ? A, r ? R, r / 0. there exists
an element b ? A such that a = rb.
As an obvious example, we find that, every divisible Abelian group is
a divisible Z-module. A submodule of a divisible -ff-module need not be
divisible. For example, the additive group Q of rational numbers is a di-
divisible Z-module but Z is not a divisible Z-module. The group of rational
numbers is a special case of the following.
Let R be an integral domain and Q be its field of quotients. Any element
of Q is of the form r/s, where r,s ? R. s / 0. These fractions have the
property that, ra/sa = r/s for every a ? R, a / 0. Therefore, for every
r/s ? Q; a ? R. a / 0. the element, r/as ? Q and a(r/as) = r/s. Thus Q
is a divisible -ff-module.
Definition 3.3.8 If R is an integral domain, an _R-module A is called
torsionfree if ra = 0. r ? R. a ? A implies either r = 0 or a = 0.
We need the following result while studying Dedekind domains (cf.
Chapter 8)
Proposition 3.3.9 Let R be an integral domain and Q be its field of quo-
quotients. Then a torsionfree R-module M is divisible if and only if M is a
vector space over Q.
Proof. Suppose that M is a vector space over Q. Let m ? M and
r ? R,r / 0. As 1/r ? Q. therefore (l/r)m = m' ? M. Then rm' =
r((l/r)m) = (r/r)m = m. Thus M is a divisible -ff-module.
Now. suppose that M is a torsionfree divisible -ff-module. Then for
every r ? R, r / 0 and m ? M, there exists a unique element m' ? M
such that m = rm'. We then define (l/r)m = m!. For r/s ? Q, m ? M,
define (r/s)m = r((l/s)m) = rm'. where m! ? Mis the unique element,
with m = sm,'. Let r/s, r'/s' ? Q. m,nii,m2 ? M. Let m!,m\,m'2 ? M
be uniquely determined elements satisfying
m = s'm'. mi = sm[. m? = sm'2.
Let m" ? M be the uniquely determined element satisfying m' = sm".
But then m = ss'm" so that (l/ss')m = m" = A/s)m' which imply that
sm" = A/s')m. Therefore
ip G _|_ G<p 1
(r/s + r'/s')m = m=(rs' + sr')(—m)
ss' ss'
= (rs' + sr')m" - r.s'm" + sr'm"
= r(—m)+r'(—m) = (r/s)m+(r'/s')m:
s s
© 2003 by CRC Press LLC
and
{{r I s){r' I s'))rn = (rr' / ss')m = rr' (—-m) = rr'm" = rr''(—m')
rr' rr' 1 . r r'
= —m = —(—m) = -(— m).
s s s' s s'
Again
nil + rn-i = sm[ + srn'2 = s(m'1 + m'2)
which implies that.
-(nil + rn.2) = m[ + rn!2.
s
Therefore
r/s(nii + m2) = r(m\ + m'2) = rm\ + rm'2
= r((l/s)mi) + r((l/s)m2) = (r/s)mi + (r
Hence M is a vector space over Q. q
Theorem 3.3.10 Every injective R-module is a divisible R-module.
Proof. Let M be an injective left -ff-module. Let m ? M and a ? R.
a not a zero divisor in R. Then Ra is a non-zero left ideal of R. Let
/ : Ra —> M be the map defined by f(ra) = rm. r ? R. Since a is not a
zero divisor in R. every element of Ra can be uniquely written as ra, r ? R
and. therefore. / is indeed a well defined map. The map / is clearly an R-
homomorphism and the module M being injective. it follows from Baer's
criterion that / can be extended to an _R-homomorphism g : R. -»¦ M'.
Therefore
m = f(a) = g(a) = g(a.l) = ag(l) = am',
where m! = g(l) ? M. Hence M is a divisible _R-module. rj
Not every divisible _R-module is an injective module. For example :
(a). Consider R = Z/AZ the ring of integers modulo 4. The only non-zero
divisors of R. are 1 + 4Z and 3 + 4Z. Consider M = 2Z/4Z = {0, 2 + AZ}
which is an ideal of R. Clearly M is a divisible -ff-module. Tf M were
an injective -ff-module. then there exists an _R-homomorphism g : R —»¦ M
which extends the identity map : 2Z/4Z -> M. This shows that. 2Z/AZ is
a direct, summand of Z/4Z which is not true.
(b). If R = Z/12Z is the ring of integers module 12. then 2Z/12Z is a
divisible i?-module but is not a direct summand of R. Corollary 3.6 then
shows that this is not an injective -ff-module.
However, for torsionfree -ff-modules. the two concepts coincide. Also if
the ring R is a principal ideal domain, the two concepts coincide.
© 2003 by CRC Press LLC
Theorem 3.3.11 Let R be an integral domain. Then a torsion-free R-
module is divisible if and only if it is injective.
Proof. Since every injective module is a divisible module, we only need
to prove that every torsion-free divisible -ff-module is injective.
Let M be a torsion-free divisible -ff-module. Let T be an ideal of R.
and / : T -»¦ M be an _R-homomorphism. Tf T = {0}. then the zero map
: R —»¦ M extends /. Therefore we may suppose that. / ^ {0}. The R-
module M being torsion-free and divisible, for every a ? I. a/ 0. there
exists a unique element ma ? M such that f{a) = ama. For a,b ? I. ab ^ 0.
f(ab) = af(b) = abmt,
and also
f(ab) = f(ba) = bf(a) = bama.
Therefore ab(mi> — ma) = 0 and ab being non-zero, we have ma = nib = m
(say). Define a map g : R —> M by g(r) = rm. r ? R. The map g is an
_R-homomorphism and for any a ? I,
g(a) = am = f(a)
showing that g extends the i?-homomorphism /. Hence M is injective. rj
Theorem 3.3.12 Let R be a principal ideal domain. Then an R-module
is divisible if and only if it is injective.
Proof. Since every injective -ff-module is divisible (Theorem 3.10). we
only need to prove that every divisible -ff-module is injective. Let M be a
divisible _R-module. Let / be an ideal of R. Then there exists an element.
a ? I such that. / = Ra. Let /:/—>¦ M be an _R-homomorphism. Since
M is a divisible -ff-module. there exists an element m ? M such that
f(a) = am. Define a map g : R. -»¦ M by g(r) = rm. r ? R. Then g
is an _R-homomorphism and for any b ? I. b — ra for some r ? R. and. so.
g(b) = bm = ram = rf(a) = f(ra) = f(b).
Thus g extends the i?-homomorphism /. Hence M is an injective .R-module.
?
Corollary 3.3.13 An Abelian group A is divisible if and only if A is an
injective Z-module.
Since there do exist torsion divisible Abelian groups for example (a)
the Prufer group Z(poo), (b) the additive group Q/Z of rational numbers
modulo 1; there exist injective modules over a principal ideal domain which
are not torsion-free.
We close this section with another simple application of Baer's criterian.
© 2003 by CRC Press LLC
Let m be an integer > 2 and R = Z/mZ. Any ideal of R is of the
form kZ/mZ where k is divisor of m. Let m = ks. Let / : kZ/mZ —> R.
be any _ff-homomorphism. Suppose that f(k + mZ) = a + mZ. Then
0 = f(ks + mZ) = sf(k + mZ) = sa + mZ which implies that m divides
sa and. therefore, k divides a. Let a = kb. Define g : Z/mZ —> Z/mZ by
g(n + mZ) = nb + mZ. n ? Z. The map g is an _R-homomorphism and
extends the map / to R. Hence R. is an injective -ff-module.
Not every ring R. is an injective -ff-module. For example the ring Z is
not an injective Z-module.
3.3.14 Exercises
1. Is the converse of corollary 3.6 true? Explicitly, if a left ideal of R. is
a direct, summand of R. is / an injective -ff-module ?
2. In the ring ZjYlZ of integers modulo 12. decide if the ideal
(a) 3Z/12Z is an injective -ff-module.
(b) 4Z/12Z is an injective -ff-module.
Observe that both of these are divisible -ff-modules and are direct sum-
mands of R.
3. Prove that, every homomorphic image of a divisible _R-module is
divisible.
4. Prove that direct sum of modules is divisible if and only if every
direct summand is divisible.
5. If {Mi,n3i}ijei is a direct, family of divisible _R-modules over a di-
directed set I. prove that lim^{Mi,nf} is a divisible -ff-module.
(The converse of the above result is not true, in general. For example,
the additive group Q of rational numbers is a divisible Z-module. Since ev-
every Abelian group is direct limit of its finitely generated subgroups directed
by inclusion. Q is direct limit of its finitely generated subgroups. But no
finitely generated Abelian group is divisible.)
6. If a left ideal T of R. is an injective -ff-module then T is a projective
i?-module (prove it. !). Is the converse true ? Justfy.
3.4 An Embedding Theorem
A central idea in homological algebra is the study of derived functors of
certain additive functors. Study of derived functors depends upon the ex-
existence of projective and injective resolutions of modules. For the existence
of projective resolutions one needs to know that every module is homomor-
homomorphic image of a projective module (this result has already been obtained)
while for injective resolutions one needs to know that every module can be
embedded in an injective module. The aim of this section is to obtain this
result. Tn the process we also get some inter-relations between Horn and
tensor product and these results are of independent interest, as well.
Let S be another ring.
© 2003 by CRC Press LLC
Definition 3.4.1 If A, B are (R, S) bimodules. a map / : A -»¦ B is called
a bihomomorphism if / is both an B. as well as S-homomorphism.
If A is an (R, S) bimodule with A a left _ff-module and right S-module.
we express it. by writing A ? rMs-
Lemma 3.4.2 If A ? Mr, R ? rMs and C G Ms, then
(i) A ®r B can be given the structure of a right S-module and
(ii) Horris(B, C) can be given the structure of a right R-module.
Proof, (i). Let s G S. Define a map s* : A x B ->¦ A ®r B by
s*(a, b) = a ® Fs). a ? A. b G B.
It is clear that, s* is biadditive. Also, for a ? A, b G B. r ? R.
s*(ar,b) - s*(a,rb) = (ar) ® (bs) - a® (rb)s
= (ar) $5 Fs) — a (g> rFs)
= (ar) (g; Fs) - (ar) <& Fs) = 0.
Hence s* is -R-bilinear and induces a homomorphism s* : A®RB -»¦ A®RB.
s*(a<Z>b) = a® Fs). a G A, b G B.
Define (a <& 6)s = s*(a ®b). a € A, b G B. With this action. A ®R B
becomes a right 5-module.
(ii) For / G Homs(B, C), r G R, define fr : B -> C by
= f(rb),b?R.
The map fr is clearly additive. Also, for b ? B, s ? S,
(fr)(bs) = f(r(bs)) = f((rb)s) = f(rb)s = (fr)(b)s.
Therefore fr is an S-homomorphism. With this action Horns(B,C) be-
becomes a right _R-module. rj
Theorem 3.4.3 If A ? Mr, B ? RMS and C ? Ms, then
Homs(A (g)/j B,C) and Hornr(A, Horns(B,C)) are canonically isomor-
phic.
Proof. For an / G Homs(A ®RB,C), define / : A -> Homs(B, C) by
(f(a))(b) = f(a ® 6). a ? A, b ? B.
For a ? A, f(a) is clearly additive. Also, for a ? A, b ? B, s ? S,
G(a))(bs) = f(a ® Fs)) = f((a ® 6)s) = f(a ® 6)s = (/(a)F))s.
© 2003 by CRC Press LLC
Thus / ?Homs(B,C)_L
From the definition of / it is clear that / is additive. For a ? A. r G R. b ?
B,
(f(a)r)(b) = J(a)(rb) = f(a®rb) = f((ar) <E> b) = (f(ar))(b).
Therefore f(a)r = f(ar) for all a ? A. r ? B. Hence / is an R-homomorphism.
Define a map
6 : Homs{A ®R B, C) -> HomR(A, Homs(B, C))
by
= f; f?Homs{A®RB,C).
It is easily seen that. 6<(/i + f2) = h + h = h + h = &(fi) + 0{f2) for
/i. J2 ? Horns (A ®rB,C) and 6 is a homomorphism.
On the other hand, for g G Homn(A, Honis(B, C)). define g : AxB ^>-
C by g(a, b) = (g(a))(b), a?A.,b?R.
The map g is clearly biadditive. Also, for a ? A, b ? B. r ? R.
g(ar,b) - g(a,rb) = (g(ar))(b) - (g(a))(rb)
= (g(a)r)(b) - g(a)(rb)
= g(a)(rb)-g(a)(rb) = O.
Therefore g induces a homomorphism
g : A <E>R B ->¦ C.
For a G A. b G B and s G S.
g((a <S) b)s) = g(a ® Fs)) = g(a)(bs) = (g(a)(b))s = g(a <g> b)s.
Thus g is an S-homomorphism.
Define 0 : HomR(A,Homs(B,C)) -> Homs(A®RB,C) by
0C) = 9, 9 e HomR(A,Homs(B,C)).
It is fairly easy to check that for g1, 52 ? HomR(A, Honis{B, C)). cp(gi +
32) = 0Ci)+ 0C2)-
Let / G Homs(A <g>R B,C). Then, for a G A: b G B.
which implies that / = / i.e. <f>8(f) = f. Therefore cpd = identity map on
Homs(A <E>R B,C). Similarly 9<p = identity on HornR(A,Horns(B,C)).
Therefore 0 is an isomorphism with 0 as its inverse.
We next prove that. 6 and 0 are functorial hommorphisms. Let A, A' G
Mr.. B,B' G rMs: C G Ms and a : A' -> A, 7 : C -> C" be
© 2003 by CRC Press LLC
module homomorphisms. /3 : B' —>¦ B a bihomomorphism. Then we get
homomorphisms a ® /3 : A' ®R B' —»¦ A ®R B.
,j) : Horns{A®RB,C) -> Homs(A' ®RB',C):
) : Homs(B,C) -> Homs(B',C):
Hom(a,Hom(P,y)) : HomR(A,Homs(B,C)) -> HomR(A',Homs(B',C')
For notational convenience we write Hom(/3,y) as (/?, 7) etc. For any
f G Horns (A ®RB,C),
C.7)
(a, (/?, i)HU) = (a, (/?, 7))/ = (/?, 7)/a
and
C.8) 0(a «) /?, 7)/ =
For «' G A', ft' G S'.
and
, 7)/
= lf(a(a')
= ((/?,7)/a(
= hf(a(a')) (/?(&'))
= 7/(a(a') ® /?F'))
Therefore
which imply that 7/(a ® /?) = (/?, 7)/a showing that the diagram
(a ®/3,7)
HomR(A, H orris (B,C))
*,@,7))
HomR{A' ,Homs{B'\C'))
is commutative. Hence # is a functorial isomorphism. That 0 is functorial
follows on similar lines, q
Lemma 3.4.4 If A G sMR. R G i?.M and C G
(i) A($r B can be, given the structure of a left S-module; and
(ii) Horns (A, C) can be given the structure of a left R-module.
© 2003 by CRC Press LLC
Proof, (i) For s ? S, define a map s* : A x B ->¦ A ®ff Z? by
s*(a, 6) = (sa) ® 6. a ? A. b ? B.
The map s* is clearly bilinear and induces a homomorphism s* : A®rB -»¦
A (g>ij S. s*(a ® b) = (sa) ® b. where aeA ft G B. For a G A b ? B and
s ? S. define
s(a (gib) = s*(a (gib) = (sa) <E> b.
This 5-action makes A ®_r B into a left 5-module.
(ii) For / G Homs(A,C), r ? R. define a map rf : A -> C by (r/)(a) =
f(ar), a ? A. The map r/ is additive and for a ? A, s ? S.
(rf)(sa) = f((sa)r) = f(s(ar)) = sf(ar) = s(rf)(a).
Thus rf is an 5-homomorphism. This R-action makes Honis(A,C) into a
left _R-module. rj
Theorem 3.4.5 If A ? sMr. B? RMandC? SM, then Homs(A®R
B,C) and Horn r(B,H orris (A, C)) are canonically isomorphic.
Proof. For an / G Homs(A ®_r B, C). define / : B -> Homs(A, C) by
(J(b))(a) = f(a®b), a?A.,b?B.
The map f(b) is additive and for a ? A. s ? S.
7F)(sa) = /((sa) ®b) = f(s(a ® b)) = sf(a ® 6) = sf(b)(a).
Therefore /F) G
That / is additive is clear. For a ? A, b ? B. r ? R.
J(rb)(a) = f(a ® rb) = f((ar) ® b) = J(b)(ar) =
which implies that. f(rb) = rf(b) for every r ? R,b ? B. Thus. / is an R-
homomorphism. Define 0 : Homs(A ®R B, C) -»¦ Hornn(B, Horns(A, C))
by
= f-, f?Horns(A®RB,C).
That. 0 is a homomorphism is easy to see.
Let g ? HomR(B,Homs(A,C)). Define g : A x B -> C by
g(a, b) = g(b)(a). a? A, b? R.
g is biadditive and for a ? A. b ? B. r ? R.
g(ar,b)-g(a,rb) = g(b)(ar) - g(rb)(a)
= (rg(b))(a)-(rg(b))(a)=O.
© 2003 by CRC Press LLC
Therefore g is i?-biadditive and it induces a homomorphism g : A($rB —> C.
g(a <g> b) = g(b)(a). a? A. be R.
Define 0 : HomR(B, Homs(A, C)) -> Homs(A ®R B, C) by
0C) = 9, 9 e HomR(B,Homs(A,C)).
For a G A. 6 G S.
g(b)(a)=g(a®b) = g(b)(a)
which implies that g = g i.e. Q(f>(g) = g. Hence ^0 is the identity map on
HomR(B,Homs(A,C)). Let / G Homs(A®RB,C), aG A, bG B. Then
7(a ® b)=J(b)(a) = f (a ® 6)
which implies that / = / i.e. 0^(/) = /. Hence 0^ is the identity map on
Horns (A ®rB,C). Therefore 6 is an isomorphism with 0 as its inverse.
Let A, A' G s^fii, ?,?' G RM,C, C G s>f and /3 : B' -> B, 7 :
C —>¦ C" be module homomorphisms and a : A' —> A be a bihomomorphism.
Then we get module homomorphisms
a <g> /3 : A' ®R B' -> A ®R B.
: Horns{A®RB,C) -> Homs(A' ®RB',C):
a,7) : Homs(A,C) -> Homs(A',C):
: HomR(B,Homs(A,C)) -> HomR(B',Homs(A',C')
As before, for notational convenience, we write (a, 7) for Hom{a,rj) etc.
For any / G Horns(A <E>R B, C),
(/?, (a, 7))fl(/) = (/?, (a, 7)O = (a, 7)//?
and
6{a ®
Now. for a' G A', ft' G B'.
C.9) 7/(a ® P){b'){a') = 7/(a <8> /?)(a' ® 6') =
and
C.10) ((a, 7)//?) (&')(«') = ((a,7OG8(&')))(a')
= 7/(a(a') ®
Relations C.9) and C.10) together imply that
or
for every / G Homs{A <%>R B, C) which proves that, the diagram
© 2003 by CRC Press LLC
Homs(A®RB,C)
(a®/?, 7)
Hams(A'®RB',C)
HomR(B,Homs(A,C))
HomR(B',Homs(A',C'))
is commutative. Hence (9 is a functorial homomorphism. Similarly. <j> is also
a functorial isomorphism, q
Theorem 3.4.6 // D is a divisible Abelian group, Hom(R,D) is an injec-
tive left R-module.
Proof. Recall that we write Hom(A,B) for Homz(A,B). Since R. G
rMr. Hom(R,D) becomes a left i?-module under the action defined by
(cf. Lemma 4.4) :
(sf)(r) = f(rs), f G Hom(R,D), r,s?R.
To prove that. Hom(R, D) is an injective i?-module. it. is enough to prove
that if A ¦% B is a monomorphism of left i?-modules then
Homn(B,Hom(R,D)) A- Homn(A, Hom(R, D)) is an epimorphism. So.
let A ¦% B be a monomorphism of left i?-modules. Consider the diagram
HomR(B,Hom(R,D))
HomR(A,Hom(R,D))
Hom{R®RB,D)
A ® a, 1)
Hom{R®RA,D)
C.11)
Hom(B,D)
Hom(A, D)
in which the upper square is commutative, the isomorphism ^ being func-
functorial (cf. Theorem 4.5). Also, for any left i?-module M. R®RM = M,
the isomorphism being functorial. Therefore the lower square in the dia-
diagram C.11) is also commutative with the lower vertical maps isomorphisms.
© 2003 by CRC Press LLC
Every divisible Abelian group being an injective Z-module. the homomor-
phism (a, 1) : Hom(B,D) —> Hom(A,D) is an epimorphism. All the four
vertical maps in the commutative diagram C.11) being isomorphisms, it
follows that (a, 1) : HomR(B,Hom(R,D)) -> HomR(A,Hom(R,D)) is an
epimorphism. Hence Hom(R,D) is an injective left i?-module. q
We still need a simple observation about. Abelian groups to prove the
main theorem of this section.
Let G be an Abelian group. Then G being a Z-module. there exists a
free Z-module -F(say) and a subgroup A of F such that F/A = G. Now
F = (Q^Z and every copy of Z is embedded in Q the additive group
of rationals. Therefore G is embedded in (®^2Q)/A. Since direct, sum
of divisible groups is divisible and Q is a divisible Abelian group. © Y Q
is divisible and then (®^2Q)/A is divisible. Hence G is embedded in a
divisible Abelian group (© Y Q)/A. We have thus proved
Proposition 3.4.7 Every Abelian. group can be embedded, in a divisible
Abelian group.
Theorem 3.4.8 Every R-module can be embedded in an injective R-module.
Proof. Let A be a left i?-module. By Proposition 4.7. there exists a
divisible Abelian group D and a group monomorphism a : A -»¦ D. For
a G A. define fa:R->Aby fa(r) = ra. r ? R. The map fa is clearly a
homomorphism of groups. The map a being one-one, we can regard Ira a
as a left R-module by defining ra(a) = a(ra). r G R. a G A. Let a G A.
For any r G R,
(afa)(r) = afa(r) = a(ra) = ra(a) = /Q(aH)-
Therefore afa = fa(a) for every a G A. Define a map 0 : A —> Hom(R,D)
by 6{a) = afa, a G A. For a, b G A, r,sG R,
fa+b(r) = r(a + b) = ra + rb = fa(r) + fb(r) = (fa + fb)(r)
and
(sfa)(r) = fairs) = (rs)a = r(sa) = fsa(r).
Therefore
0(a + b) = afa+b = a(fa + fb) = 6{a) + 6{b)
and
6{sa) = afsa = fa(sa) = fsa(a) = sfa(a) = s(afa = s6(a).
Hence 0 is an i?-homomorphism. If 0(a) = 0, then fa(r) = 0 for every r G R.
In particular /a(l) = 0 i.e a = 0. This proves that. 9 is a monomorphism.
?
© 2003 by CRC Press LLC
Chapter 4
Homology of Complexes
Our aim in the present chapter is to introduce what is called connecting
homomorphism using which we can obtain a long exact sequence corre-
corresponding to a given exact sequence of complexes. First a special case of the
connecting homomorphism is defined using which the kernel-cokernel exact
sequence is obtained. The concept of homotopy is introduced.
4.1 Ker-Coker Sequence
Recall that we write H(A — B - C) for the homology of the complex A -»¦
B —> C of i?-modules and homomorphisms i.e. for Kerg/Imf.
Lemma 4.1.1 Given a commutative diagram
a\ 0L2
h
of R-modules and homomorphisms with the rows as O-sequences, there exists
a homomorphism H(f) : H(AX — A2 — A3) —> H(Bi — B2 — B3). Moreover
the homomorphism is natural.
Proof. While considering examples of categories, we have already proved
the existence of the homomorphism H(f) : H(AX — A2— A3) —> H(Bi —B2 —
the existence of the homomorphism H(f) : H(Ai A2A3) > H(Bi B
S3). Recall that H(f)(a,2 + Imai) = f2@,2) + ImPi-. where a^ G Ker ai-
For proving the naturality of the homomorphism. consider a (three-
dimensional) commutative diagram with rows as O-sequences :
97
© 2003 by CRC Press LLC
We then have four B dimensional) commutative diagrams
A
As A
A>
B,
B'
B'
B>
A>
B'2
A'
with rows as O-sequences and the homomorphisms as indicated in the 3-
dimensional diagram above. These diagrams induce homomorphisms
H(f) : H(AX -A2- As) ->¦ H(B1 - B2 - B3)
H(j) : ff (A -A2- As) -»¦ H(A[ - A, - As)
HF) : H(Bl -B2- B3) -> H(B[ - B2 - B1^) and
H{f_) : H{A\ -A>2- A>3) -> H{B\ - B'2 - B'3).
For any a2 G Kera2.
(H(S)H(f))(a2 + Im(ai)) = H(S)(H(f)(a2
© 2003 by CRC Press LLC
S2(f2(a2)) + Im p[
(S2f2)(a2)+ImP[
= H{f){l2{a2)+Ima\)
This proves that HF_)H(f) = H(f')H(j) so that the diagram
(A, -A2- A3)
H(f)
i — B2 —
H(A[ -A!2- Atf
H(f')
H(B[ -B2-
is commutative. This completes the proof that the homomorphism H(/)
H(Ai — A2— As) —> H(Bi — B-2 — -B3) is natural ( or functorial). q
Corollary 4.1.2 Given a commutative di
A
a
B
A'
B'
of R-modules and homomorphisms, there exist natural homomorphisms a :
Ker f —> Ker g and a' : Goker f —> Goker g.
Proof. The existence of natural homomorphism a : Ker f —> Ker g
follows from Lemma 1.1 by considering the diagram
© 2003 by CRC Press LLC
A
-*¦ A'
B
B>
9
and the existence of natural homomorphism a' : Coker f -»¦ Coker g follows
from Lemma 1.1 when we consider the commutative diagram
A
f
A'
0
a
B
¦*¦ B'
Explicitly, the homomorphisms a and a' are defined as follows
a(a) = a(a). a G Ker f,
and
a'(a' + Imf)=a'(a')+Img, a' G A'u
Proposition 4.1.3 Given a commutative diagram
A f3 H 93 n n
A3 «- -D3 «- O3 «- U
73
R
B-2
92
a2
72
h
B^
9\
of R-modules and homomorphisms in which the rows are exact and the
columns are O-sequences, then there exists a natural exact sequence
D.1) H(A3-A2-Al)
H(f)
H(g)
H(C3 - C2 -
© 2003 by CRC Press LLC
Proof. That, the required sequence is natural follows from the homo-
homomorphisms H{f) and H{g) being natural (Lemma 1.1). We. therefore,
only need to prove exactness of the sequence D.1).
Let a2 G Kera2. Then
H(g)H(f)(a2 + Ima3) = H(g)(H(f)(a2 + Ima3))
= H(g)(f2(a2)+Imp3)
= 52(^@2)) + /m73 = 0. as Imf2 = Ker g2.
This proves that Im H(f) C KerH(g). Let 62 G Ker (i2 such that.
H(g)(b2 + Imj33) = 0. Then 52F2) = 73@3) for some c3 G C3. Let
63 G B3 such that c3 = 53F3). Then
52F2) = 73 (c3) = G353) F3) = E2/^3) F3)
which implies that, there exists an a2 G A2 with 62 = ^3F3) + f2(a2). Now
/ia2(o2) = ^2/2@2) = /32F2 - /33F3)) = /32F2) = 0.
The map fi being a monomorphism. we get 0:2@2) = 0 i.e. a2 G Ker a2.
Also
H{f){a2 + Ima3) = f2(a2) + Imf33 = b2 - /33F3) + Imf33 = b2 + Imj33.
This proves that KerH(g) C ImH(f) and. hence. ImH(f) = KerH(g)
and the sequence D.1) is exact, q
Theorem 4.1.4 Given a commutative diagram
A * B ? * C >- 0
f
9
h.
A' B' *¦ C"
a' /?'
of R-modules and homomorphisms with exact, rows, there exists a natural
exact sequence
Ker f A Ker g —> Ker h. —)¦ Coker f ^> Coker g —$¦ Coker h.
Proof. Existence of homomorphisms a,/3,a',/3' follows from Corollary
1.2. We next define a homomorphism A : Ker h —> Coker f.
Let c G Ker h. As f3 is an epimorphism. there exists an element b G R such
that c = /3F) . Then
0 = h(c) = h(P(b)) = (W) = W'9)(b) = P'(g(b))
© 2003 by CRC Press LLC
so that. g(b) G Ker /?' = Imcr'. Since 01' is a monomorphism. there exists
a unique element a' G A' such that a'(a') = g(b). Let b\ G B be another
element such that P(b{) = c. Let a[ G A' such that a'(a'i) = g(bi). Then
/?(&i — b) = 0 and there exists an element a G A such that b\ — b = a(a).
Therefore
9(h) ~ 9(b) = g(h -b)= ga(a) = a'f(a)
so that
a'(a[)-a'(a') =a'f(a)
and a' being a monomorphism. a[ — a' = f(a) which implies that a[ +
Ira f = a' + Imf. This proves that the element a' + Ira f is uniquely
determined.
Define A : Ke.r h. —> Coker f by
A(c) = a' + Iraf. cGKerh.
where a' G A' is obtained by c = /?(&). b G B, and g(b) = a'(a1).
Let Ci,C2 G Kerh and r, s G i?.. Choose &i,&2 G /? and a'^a^ ? ^' such
that.
Ci=/?(&i): »F0 = a'(oj), i = l,2.
Then
A(Ci) = 4 +Imf, i = 1,2.
Now. iffir /;. being a submodule of C. rc\ + SC2 G ifsr h. and
rCl+sc2 = r/?(&!) + sj3(b2) = Pirbi) + P(sb2)
g(rbi+sb2) = rg(bi) + sg{b2) = ra1\a[) + so1\a2)
= a'(ra\) + a'(sa'2) = a'(ra\ + sa'2)
Therefore
A(rci + sc2) = ra[ + sa'2 + Ira f
= r(a[ + Im f) + s(a'2 + Im f)
= rA(d)+sA(c2).
Hence A is an i?-homomorphism. Exactness of the sequences
D.2) Ker f 4 Kerg A Kerh
and
D.3) Coker f ^ Coker g ^ Coker h
follows from Proposition 1.3.
© 2003 by CRC Press LLC
Let b G Kerg. Then /?(&) = c G Kerb, so that, c = /?(&) and 5F) = 0 =
a'@) and it follows from the definition of A that A(c) = 0 + /m / = 0 i.e.
AC(b) = 0 which shows that Im~P C KerA.
Next, let c G Kerh. Choose ft e B and a' G A' such that /?(&) = c
and 5F) = a'(a1). Then A(c) = a' + Imf. Suppose that A(c) = 0. Then
a' = /(a) for some a G A. Now
»(&)=a'(a')=a7(a)=0a(a)
and. so.
g(b - a(a)) = 0 i.e. b - a(a) G Ker g
and
0F - a(a)) = 0(b - a(a)) = /?(&) = c.
This implies that KerA C IraE and. hence, /m/? = KerA. This com-
completes the proof of the exactness of the sequence
D.4) Ker g A- Ker h -»¦ Coker f
Next consider the sequence
D.5) Ker h. -»¦ Coker f % Coker g.
Let c G Kerh. Chose b ? B and 0/ G A' such that. /?(&) = c and
g(b) = a'(a1) so that. A(c) = a' + Imf. Then
a'(o' + Im f) = a'(a') + Img = g(b) + Img = 0
which shows that /m A C Ker a'.
Now, let a' G A' such that a'(a' + Im f) = 0 i.e. a'(a') + /m g = 0.
Therefore, there exists b G R such that a'(o') = g(b). Put /?(&) = c. Then
fc(c) = ^F) = P'g(b) = 0'a'(a') = 0
and c G Kerh. Since c = /?(&) and g(b) = a'(a') already, we have A(c) =
0/ + Im f. Thus Kern' C 7mA and. so. 7mA = Kern'. This proves that.
the sequence D.5) is exact. Combining the four exact sequences D.2) to
D.5). we get an exact sequence
Ker f —> Ker g —> Ker h —> Coker f —)¦ Coker g —)¦ Coker h.
Since the homomorphisms a. /?. a'. /3' are all natural, in order to prove that
the above sequence is natural, it is enough to prove that the homomorphism
A is natural. For this we consider a (three dimensional) commutative
diagram
© 2003 by CRC Press LLC
A
*¦ N'
Let c G Ke.rh. Choose b G B and a! G A' such that. /?(&) = c and
g(b) = a'(a'). Then A(c) = a' + /m/. Now fci/(c) = v'h(c) = 0 so that
i'(c) G ATer fc. Also /j,(b) G M and A'(a') G V such that
= !//?(&) = !/(C)
and
and the definition of A shows that.
AF(c) = A'(a' + Imf) = A'A(c).
This proves that, the diagram
Ker h.
A
Ke.rh,
Coker f
A'
Coker i
A
is commutative and the homomorphism A is natural, q
The exact sequence of Theorem 1.4 is called Ker-Coker sequence.
Also the homomorphism A is called the connecting homomorphism.
© 2003 by CRC Press LLC
4.2 Connecting Homomorphism-the General
Case
We consider a more general situation than the one considered in the section
above. The result of Theorem 1.4 then follows as a special case. Consider
a commutative diagram
0
03
h „ 02
c2
a2
02
0i
D.6) 0
/o
of i?-modules and homomorphisms with exact rows and the columns as 0
- sequences. Let c2 G Ker 72. There exists an element. b2 ? B2 such that.
Ci = 52(^2) and
= 0.
Therefore there exists an element, cjj G Ax such that. f\{a\) = ^2F2)- Now
/Oai(o!) = ^1/1 (oO = 0i@2(b2)) = 0
and /o being a monomorphism ai(ai) = 0 i.e. a-\ ? Kera\. To sum up.
we have proved that for c2 ? Ker 72; there exist. b2 ? B2. a\ ? Kera.\ such
that.
D.7) c2 = 52(^2) and /^oi) = #2F2).
Let b'2 ? B2. a[ ? Ker ai be another pair of elements such that
D.8) c2 = fl2 (b'2) and frfa) = 02(b'2).
Then g2(b'2 - b2) = 0 i.e. b'2 — bi G Ker Qi = Im /2 and there exists a2 G ^2
such that b'2 - b2 = f2(a2). Therefore
hia'.-a,) = f1(all)-fl(al)=C2(b'2)-P2(b2) = p2(b'2-b2)
= I32f2(a2) = fia2(a2)
© 2003 by CRC Press LLC
which implies that.
a[ — a-\ = cx2(a2) and. so. a[ + Ima2 = at + Ima2.
This proves that the element at + Im u2 is independent of the choice of
62 G B-2 and at G Kerat satisfying D.7). Next, let c2 G Ker 72 such that
c2+/m 73 = C2+/m73. Then there exists C3 G C3 such that c2 = c2 +73@3).
Let 63 G -B3 such that. 33F3) = C3. We have
c'2 = c2 + 73(c3) = 52F2) + 7353(^3) = 52F2) + 52^3F3) = 52F2 + ^3F3))
and
I32(b2 + /?3F3)) = ?2F2) + /?2/?3F3) = ?2F2) =
It. therefore, follows that the element at + Ima2 G H(A2 - At - Ao) is
independent of the choice of the representative C2 of the element C2+/m73 G
H(C3 -C2-Ct). Define a map
A : H(C3 -C2- d) ->¦ ff(^2 - A1 - Ao) by
A(c2 + Im^z) = at + lma2.
where 62 G Bi and a\ G -ftTer ai have been chosen for c2 G -ftTer 72 to satisfy
D.7).
Let C2,c2 ? ifer72 and r, s G R. Choose 62,62 G B2 and ai, a'x G Kerat
such that c2 = 52F2): c2 = 52 F2) and /32 F2) = /i(oi): /32F2) = AK) so
that
A(c2 + Im73) = ai + lma2. A(c2 + Im^3) = a[ + Ima-2.
Now
rc2 + sc2 = 7-32F2) + S52F2) = 52G-62 + s62)
and
/?2(r62 + sb'2) = r/32(b2) + sC2(b'2) = r/i(Ol) + s/iK) = ft(rat + sa[
Therefore
A(r(c2 + Im 73) + s(c2 + Im 73)) = A(rc2 + sc2
= rat + sa\ + Im a2
= r(at + Im a2) + s(a[ + Im a2)
= rA(c2 + Im73) + sA(c2 + 177173)
and. so. A is an _R-homomorphism.
The homomorphism A constructed above is called the connecting ho-
homomorphism.
We next prove that
© 2003 by CRC Press LLC
Proposition 4.2.1 The. connecting homomorphism A is natural.
Proof. Consider a (three dimensional) commutative diagram with exact
rows and the columns as O-sequences :
0
fl3
(f>3
h2
Ms 1
03
A fl
A1 —
a1 i
H2 1
Bl
9\
Mi-^i
/o
72
Let C2 G ifer72. Choose 62 G -^2, ai G Kera.\. such that 32(^2) = C2 and
fi(ai) = ^2F2)- Then A(c2 + Im^) = ai + Ima-2.
Let n2 = rip2{c2)-. h = Oi(ai) and m2 = ^(fe)- Then
(C2) = 0;
= n2;
© 2003 by CRC Press LLC
and
hi(h) = hit
These relations together imply that
A(n2 + Im v3) = li + Im A2
or
A(i(}2(c2) + Imv3) = 9i(ai) + Im\2
or
A(HO/0(c2 + Im 73)) = H(9)(ai + Ima2) = H(9)(A(c2 + Im 73))
i.e.
+ Im 73) = (H(9)A)(c2 + Im 73)
which implies that AH{ip) = H@)A. This proves that the diagram
H(C3-C2-d) -JlML H{N3-N2-N,
A
A
H(A2 -A,- Ao) H(L2 -Lx- Lo)
H{9)
is commutative and the connecting homomorphism A is nat.ural. q
Theorem 4.2.2 Given the commutative diagram D-6) of R-modules and
homomorphisms with, exact rows and, the columns as O-sequences, the se-
sequence
H(Bi-B2-Bl) HM] H{C3-C2-C{) 4 HiA^A^Ao) "M} H{B2-Bx-B0
D.9)
is exact. Moreover, this exact sequence is natural.
Proof. That the sequence is natural follows because the homomorphisms
H{f). H(g) and A are natural. We thus need to prove that the sequence
is exact.
Consider an element b2 + Im f33 G H(B3-B2- Bx), b2 G Ker C2. Then
H(g)(b2+ImC3) = g2{b2)+Imlz. Set g2(b2) = c2. Now&fa) = 0 =/i@)
and it follows from the definition of A that. A(c2 + Im 73) = 0 + Im a2 = 0.
Thus ImH(g) C Ke.r A.
Let c2 + Im73 G H(C3 - C2 - d) such that A(c2 + Im73) = 0. Tf
b2 G B2. ai G Ker ai are such that /i(ai) = P2(b2). g2(b2) = c2. then
0 = A(c2 + Im 73) = a\ + Im a2
© 2003 by CRC Press LLC
so that, there exists a2 G A2 such that. a,\ = 02@2). Now
#2F2-/2@2)) = /?2F2)-/?2/2(a2) = /i(o1)-/1a2(o2) = /1 @0-/1@0 = 0
and
H(g)(b2 - h{ai) + Imfo) = 52(^2) - #2/2(^2) + 7m73 = c2 + 7m73.
This proves that Ker A C ImH(g) and hence ImH(g) = Ker A.
Next consider an arbitrary element c2 + 7m 73 G 77(C3 - C2 — C\). Let
62 G 7?2; ai G iferai such that c2 = gi(bi)- f5i(bi) = /i(oi)- Then
= H(f)(a1+Ima2)=f1(a1)
+ Im fc =0.
This proves that. 7m A C KerH(f).
Conversely, let. ai G Keroti such that. 77(/)(ai + Ima.2) = 0. Then
0 = /i(ai) + 7m^2 so that /i(ai) = ^2F2) for some b2 G B2. Take
C2 = 52(^2)- Then
72F2) = 7252(^2) = gikibi) = 9ifi(ai) = 0 i.e. c2 G
The relations fi{a{) = ^2F2): c2 = 32(^2) then show that A(c2 + 777*73) =
ai + Ima?,. This proves that Ker H(f) C 7mA and hence 7mA =
KerH(f). This completes the proof that the sequence D.9) is exact, q
Next we consider a very useful application of Proposition 1.3 and The-
Theorem 2.2.
Unless otherwise stated explicitly all complexes (i.e. 0-sequences) shall
be complexes of R-modules and homomorphisms.
Definition 4.2.3 Let (X,d). (X',d') and (X",d") be complexes and f =
{/„} : (X',d') -> (X,d): g = {gn} : (X,d) ->• (X",d") be chain maps.
We say that the sequence
x'-^xl x"
is an exact sequence of complexes if the sequences
are exact for every n.
Theorem 4.2.4 Given an exact sequence
0 -> X' 4> X -§> X" -> 0
© 2003 by CRC Press LLC
of complexes, there exists a long exact, natural sequence
¦""(I) TT CV\ "\S) TT fvll\ A TT fvl\
Proof. For any n. we have a commutative diagram
•VI Jn+l y~ fln+1 vll
0
d'
n+1
dn
Jn y
An
dn
+l
9n-l
n-\
X'n-V
in which the rows are exact and the columns are O-sequences. It follows
from Proposition 1.3 and Theorem 2.2 that the sequence
D.10) Hn(X')
is a natural exact sequence. Combining the exact sequence D.10) for all
possible values of n we get the desired natural exact sequence, q
Observe that a complex X is exact if and only if Hn(K) = 0 for every
n. Also a sequence 0 —> A —> 0 is exact if and only if A = 0. Using these
we immediately get
Corollary 4.2.5 Let 0 -»¦ X' -»¦ X -»¦ X" -»¦ 0 be an exact sequence of
complexes. If any two of the complexes X'. X. X" are exact, then so is
the third.
The exact sequence
¦ • ¦ -> Hn+1(X) -> Hn+1(X") 4 Hn(X') ->¦ ff
© 2003 by CRC Press LLC
is called the exact homology sequence corresponding to the exact se-
sequence 0 -»¦ X' -»¦ X -»¦ X" -»¦ 0 of complexes.
4.2.6 A complex X for which Xn = 0 for n < 0 i.e. which is of the form
•••-»¦ Xn+i -»¦ Xn ->¦ Xn-i -»¦•••-»¦ Xo ->¦ 0 ->¦ 0 ->¦ • • •
is called a left complex and we then write it as
> Xn+1 -> Xn -> Xn-i -> > Xo -> 0.
A complex X in which Xn = 0 for all n > 0 i.e. which is of the form
• • • -»¦ 0 -»¦ 0 -»¦ X_i ->¦ X_2 -»¦•••-»¦ X_n ->¦ X_n_i ->¦ • • •
is called a right complex. Also then we write it as
0 -> X° -> X1 -> > X™ -> X" -> • • •
i.e. instead of taking negative subscripts, we take non-negative superscripts.
Observe that, here X_n is being represented as X™ for every n.
If X is a left complex, then Hn(K) = 0 for all n < 0 while if X is a right
complex, then Hn(X.) = 0 for all n > 0.
Therefore, if X'. X. X" in Theorem 2.4 are left complexes, then the exact
homology sequence corresponding to the exact sequence
D.11) 0 -> X' -> X -> X" -> 0
takes the form
while if D.11) is an exact sequence of right complexes, then the correspond-
corresponding exact homology sequence takes the form
0
4
4.3 Homotopy
Definition 4.3.1 Let {X,d}. {X',d'}. be complexes and f = {/„} : X ->
X' be a chain map (or a translation), (a) The chain map f is called a
homotopy (or a null homotopy or an inessential translation) if for
every n there exists a homomorphism hn : Xn —> X^+1 such that. fn =
/in-i dn + d'n+1hn for all n.
© 2003 by CRC Press LLC
(b) If g = \gn} : X —> X' is another chain map. then the chain maps f
and g are said to be homotopic if f — g = {/„ — gn} is a null homotopy
i.e. if there exist homomorphisms hn : Xn —> X'n+l such that fn — gn =
hn-\dn+d'n+lhn for every n. The morphism h = {hn} : X -»¦ X' satisfying
the above relations is called a homotopy between f and g.
Proposition 4.3.2 Let |X,d). {X',d'| be two complexes and f — {/„} :
X —> X' be a null homotopy. Then the induced homomorphism Hn{t) = 0
for every n.
Proof. Since f = {/„} is a null homotopy. there exist homomorphisms
hn : Xn -»¦ X'n+l such that, for every n
D.12) fn = hn-Ydn + d'n+1hn
Let x G Ker dn. Then
i) = fn(x) + Im d'n+1
= (hn-idn + d'n+1hn)(x)+Imd'n+1
= hn-i(dn(x)) + Im d'n
= 0 + Imd'n+1 = 0
+1
Hence Hn(i) = 0 for every n. rj
Theorem 4.3.3 Let {X,d}. {X',d'} be complexes and f = {/„}, g =
ifln) ftfi two chain maps : X —> X'. If the chain maps f and, g are ho-
homotopic, then they induce identical maps on the homology modules i.e.
Hn(f) = Hn(g) for every n.
Proof. Suppose that f and g are homotopic chain maps. Then there
exist homomorphisms hn : Xn —> X'n+l such that
D.13) fn-9n = d'n+1hn + hn-idn for every n.
Let x ? Kerdn. Then
Hn(i)(x + Imdn+i) = fn(x) + Im d'n+1
= (gn+d'n+1hn + hn-1dn)(x)+Imd'n+1
= gn{x) + d'n+1(hn(x)) + hn-i(dn(x)) +Imd'n+1
= gn{x) +hn-i(dn(x)) +Imd'n+1
= gn{x) + Im d'n+1
= Hn(g)(x + Imdn+i).
This proves that. Hn(f) = Hn(g). Since the subscript n was chosen arbi-
arbitrarily. Hn(i) = Hn(g) for every n. rj
© 2003 by CRC Press LLC
Proposition 4.3.4 Let |X,d). {X',d'| be complexes and f = {/„). g =
{dn} be two homotopic chain maps : X —> X'. If T is an additive (covariant
or contravariant) functor rM —> nM.{orAb), then the chain maps T(f) =
{T(fn)} and T(g) = {T(gn)} are also homotopic.
Proof. Since f and g are homotopic chain maps, there exist homomor-
phisms hn : Xn —> X!n+l such that
D.14) fn-gn = d'n+lhn + hn_idn for every n.
Suppose that. T is a contravariant. functor. For every n. we have a homo-
morphism T(hn) : TX'n+l —> TXn and
T(fn ~ gn) = T(d'n+1hn + hn-xdn)
i.e.
T{fn)-T{gn) =T{d'n+lhn)+T{hn-ldn) =T{hn)T{d'n+l)+T{dn)T{hn-l)
which proves that Tf = {Tfn} and Tg = {Tgn} are homotopic chain maps.
The case when T is covariant follows on similar lines, rj
Corollary 4.3.5 Let |X,d). {X',d'| be two complexes, f = {/„) : X ->
X' a chain map and T : rM —> nAi(orAb) an additive covariant functor.
If f is a null homotopy, then T(i) = {T(fn)} : TX —> TX' is also a null
homotopy.
Corollary 4.3.6 Let X, X', be as in Proposition 3.4 and T : RM -»¦
nM.{orAb) be an additive contravariant functor. Iff is an inessential trans-
translation, then T(f) is an inessential translation : TX' —> TX.
Corollary 4.3.7 Let {X,d}. {X',d'} be two complexes, f = {/„} and
g = {<?„} be two homotopic chain maps : X —> X' and T be an additive
covariant functor. Then
Hn{Ti) = Hn(TK) : Hn(TX) -> Hn(TX')
for every n.
Let |X,d) be a complex. It is trivial that 1 = {!.„) : X -»¦ X. and
0 = {0n} : X —> X. where ln : Xn —> Xn is the identity map i.e. ln{x) = x
for every x G Xn and 0n : Xn ->¦ Xn is the zero map i.e. 0n(x) = 0 for
every x ? Xn, are chain maps. A morphism h = {hn}: where hn : Xn —>¦
Xn+i such that. dn+ihn + hn-idn = ln for every n is called a contracting
homotopy : X —> X.
Let h : X —> X be a contracting homotopy. Since 0 = \0n] : X —> X
induces the O-homomorphism : Hn(X.) -»¦ Hn(X.) for every n, it follows from
Theorem 3.3 that 1 = Hn(V) = Hn@) = 0 i.e. the identity homomorphism
: Hn(X.) —> Hn(X.) is the zero map for every n. But the identity map
\a '¦ A —> A for a module A is the zero map if and only if A = 0. Hence
Hn(X.) = 0 for every n and X is an exact sequence. Thus
© 2003 by CRC Press LLC
Proposition 4.3.8 If there exists a contracting homotopy h : X —> X, X
a complex, then X is an exact sequence.
Proposition 4.3.9 Let {X,d}; {X',d'}. {X",d"} be complexes, f =
{fn}, g = {fln} : X —>¦ X' fte homotopic chain maps and f = {fn}. g' =
{g'n} be homotopic chain maps : X' —> X". Then f'f = {fnfn} and
g'g = {flnfln} ft^e homotopic chain maps : X —> X".
Proof. Let h = {/in} : X —> X' be a homotopy between f and g and
h' = {h'J : X' -> X" be a homotopy between f and g'. Then
n ~ 9n = tin — 1 "n + "ln+l'l'n
and
for every n. For any n.
JnJn ~ 9n,9n JnJn ~ Jn9n + Jn9n ~ 9n,9n
= fn(hn-idn + d'n+lhn) + (tin_lCl!n
h'n_i (gn-\dn) + d'^
l{f'n+lhn + h'ngn)
where kn = f'n+\hn + h'ngn : Xn -»¦ X'^+1. This proves that, k = {kn} is
a homotopy between f'f and g'g : X -»¦ X". and hence f'f and g'g are
homotopic. q
4.3.10 Exercises
1. The relation of two chain maps being 'homotopic' is an equivalence
relation.
2. If X is an exact sequence, does there exist a contracting homotopy
of X ?
3. Tf f and g are two chain maps : X —> X' such that Hn{t) = Hn(g)
for every n. are f and g always homotopic ?
(The answer is no ! Example : Consider complexes X and Y, where X\ = Z
generated by x\ (say). Xq = Z generated by Xq say. and Xn = 0 for
n ^ 0. 1; d\ : Xx —> Xq is given by d\{x\) = 3xn i.e. it is the multiplication
by 3. Yt = Z generated by yx (say), Yn = 0 for n ^ 1. Then Hn(X) = 0
for n ^ 0: H0(X) = Z/3Z, i?i(Y) = Z, Hn(Y) = 0 for n ^ 1.
Let fi : Xi —> Y-\ be the homomorphism given by fi(xi) = y\. For
n ^ 1. fn : Xn -> Yn is trivially the 0-map. Then f = {/„) : X -> Y
is a chain map. We take g = \gn} : X —> Y the 0-chain map i.e. gn
© 2003 by CRC Press LLC
is the zero map for every n. Then Hn(g) = 0 for every n. Also /„ = 0
for n ^ 1. Hn{i) = 0 for every n/ 1 and iJi(X) being the 0 module,
-ffi(f) = 0. Hence Hn{i) = 0 = Hn(g) for every n. Iff and g are homotopic
chain maps, then so are Tf and Tg for any additive functor T. In particular,
this is so for the functor T = — <$S Z/3Z. Then, we have a commutative
diagram
X
0
0
Z/3Z)
Now 3 $5 1 being multiplication by 3 is the zero map. Therefore Hi (X $5
Z/3Z) = Z/3Z. Also /j being an isomorphism. fY $§ Z/3Z is an isomor-
isomorphism and hence H\(i $5 Z/3Z) : Z/3Z —> Z/3Z is an isomorphism. But
Hx(g & Z/3Z) is the 0-map. Therefore iJi(f & Z/3Z) ^ iJi(g <g; Z/3Z).
This proves that, f <$S Z/2>Z and g <g; Z/2>Z are not homotopic chain maps
and hence f and g are not homotopic.)
Use the ideas in the example above to show that the answer to Exercise
2 is also in the negative. Try the exact sequence X in which X-2 = X\ =
Z. Xo = Z/3Z, Xn = 0 for n ^ 0. 1. 2. and d2 is multiplication by 3 while
di is the natural projection.
© 2003 by CRC Press LLC
Chapter 5
Derived Functors
We have now almost, enough background material to develop methods
which are able to derive functors corresponding to a given additive func-
functor. The functors so obtained are called left or right derived functors
of a given additive functor. The study of derived functors of the functors
Homn(-,A). Homn(A, -). -®RA and B®R-. where A is left. i?-module
and B a right i?-module. is the central theme in homological algebra. It is
proved that the category of modules has enough projectives and injectives.
Comparison theorem for two projective resolutions and the corresponding
result for two injective resolutions of the same module are obtained. Left
and right derived functors of an additive functor T are defined. Long exact
seqences for LnT and RnT corresponding to a given short, exact sequence
are obtained.
Although derived functors of non-additive functors can also be defined,
we do not study those here.
Throughout this chapter, by a functor we shall always mean an additive
functor.
5.1 Projective Resolutions
Definition 5.1.1 Let A be a given i?-module. An exact sequence of the
form
E.1) P:-^Pn+1^1P^Pn_1^-4?a44^0
in which every Pn is a projective i?-module is called a projective resolu-
resolution of the module A. Also then the sequence (no longer exact at Po)
E.2) PA : ¦ ¦ ¦ -> Pn+1 H1 PnHpn_l^---^Pl%P0^Q
is called the deleted complex of the resolution P.
117
© 2003 by CRC Press LLC
We may. in fact, define the deleted complex of any complex
•••->¦ Xn+i ->¦ Xn ->•••-> X, ->¦ Xo ->¦ A ->¦ 0
in a similar fashion.
Theorem 5.1.2 Every (left) R-module A has a projective resolution.
Proof. Since every i?-module is homomorphic image of a projective
i?-module. there exists a projective i?-module Po and an epimorphism e :
Po —> A. We then get an exact sequence Po 4 A —> 0.
Let n > 0 be a given integer and suppose that we have already obtained
an exact sequence
E.3) Pn H Pn-Y -> > Po 4 A -> 0
in which every Pn is a projective R- module. Let Kn be the kernel of dn.
Then the above sequence can be extended to an exact sequence
0 -> Kn -4 Pra ^ Pra_i -> > Po 4 A -> 0.
where « is the inclusion map. As for any module Kn. being a homomorphic
image of a projective module, there exists a projective module Pn+\ and
an epimorphism 8n+\ : Pn+\ —> Kn. Take dn+\ = i8n+\. Then Imdn+i =
Im (i5n+i) = Kn = Ker dn and we get an exact sequence
Pn+l rf4X Pre ^ Pre-i ^ • • • ^ P ^ Po 4 A ^ 0
This completes induction and we get an exact sequence
E.4) ..--). Pn+1 H1 Pn H Pn_x -)¦ ¦ • ¦ % Po 4 A -> 0
in which every Pre is a projective module. Hence E.4) is a projective reso-
resolution of A. q
5.1.3 Examples
(a) Let G be a finite cycle group of order n. Then G is isomorphic to
Z/nZ. Z the additive group of integers. We thus have an exact sequence
0—)¦ Z —)¦ Z —> G —> 0 where n is the natural projection and n : Z —> Z is
the multiplication by n. We thus have a Z-projective resolution
> Pm+1 -> Pm ->¦ > P 41 Po 4 « -> 0
of f? where Po = p = Z. Pm = 0 for m > 2. e = tt and <ii is the multipli-
multiplication map by n.
© 2003 by CRC Press LLC
(b) Let G be an infinite cyclic group. Then G = Z and we may take a
Z-projective resolution
> Pn -> Pn_! -> >P, 4Po4Z40
of G. where Pq = Z. Pn = 0 for n > 1. and e is the identity map.
(c) Let G be a finite cyclic group of order n generated by x(say). Let
Z be regarded as a ZG-module under trivial G-action i.e. ya = a for every
y G G. a G Z. Let TV = YJlZo ^¦ T = x-1. Since NT = TN = x11 -1 = 0
in the integral group ring ZG of G. we get a complex
E.5) > Pm -> Pm_! 4..4p,4Poi>Z^0
where each Pm = ZG. d2m = N, d,2m+i = T1 and fi is the augmenta-
augmentation map. Define a contracting homotopy s = \sm}. where sm : Pm —>¦
Pm+i; m > 0 and s_! : Z —> Po are Z-homomorphisms which on the
elements of G are defined by
^{
1 + x + • • ¦ + xk 1. if A; > 1
0 if 0 < A; <n- 1:
It may be checked that
E.6)Ts2m + S2m-lN =
Using these relations we find that the sequence E.5) is exact and. hence, it
is a ZG-projective resolution of Z.
(d) Let G be an infinite cyclic group generated by x(say). Then
where T is the multiplication by x — 1 and e. is the augmentation map.
is an exact sequence and hence a ZG-projective resolution of the trivial
ZG-module Z.
An i?-module can. in general, have more than one projective resolution.
For example, if n is an integer > 2. then
>0->0-> > 0 -> Z -% Z 4 Z/nZ -> 0
© 2003 by CRC Press LLC
is a Z-projective resolution of Z. Also
0 -> Z®Z 4 Z®Z A Z/nZ -> 0
where a(a, b) = (na, b). and /3(a, b) = a + nZ. a, b ? Z is trivially an exact
sequence. Therefore
>0->04-04Z®z4ZffiZ^ Z/nZ -> 0
is also a Z-projective resolution of Z/nZ. Let A; be an integer > 2 with
g.c.d.(k, n) = 1. Define a map j : Z -> Z/nZ by 7@) = A;a + nZ. neZ.
The map 7 is an epimorphism and the sequence
0^2424 Z/nZ -> 0
is exact giving yet another Z-projective resolution
>0->0-> > 0 -> Z -% Z 4 Z/nZ -> 0
of Z/nZ. But any two i?-projective resolutions of an i?-module A are
related to each other.
Definition 5.1.4 Given complexes
X : > Xn H Xn_i d^ > X, ^Io4i->0
Xi . __s. V d>» Y"' ''"T1 ^ \^' d\ V1 e\ p ^ n
of i?-modules and homomorphisms. and a homomorphism / : A —> B. a
collection of homomorphisms \fn}. fn : Xn —> X'n for n > 0. is called a
chain map over the homomorphism / if fn-\dn = d'nfn for n> 1 and
e'/o = /?•
Theorem 5.1.5 (Comparison theorem) Given a diagram
Po
/bf-B- *Bn+1 * Bn Bo B 0
dn+i di ?7
of R-modules and homomorphisms in which the lower row is exact and every
Pn in the upper row is a projective R-module, there exists a chain map
f = \fn} : P/l —> /bfBB over f and two such chain maps are homotopic.
© 2003 by CRC Press LLC
Proof. We prove the existence of P-homomorphisms fn : Pn —> Bn such
that dn+\fn+\ = fndn+i for all n > 0 and fe = 77/0 by induction on n.
Since Po is a projective i?-module and r\ is an epimorphism. there exists
an i?-homomorphism f0 : Po -»¦ Bo such that r?/0 = fe. Suppose that n > 0
and that we have already constructed i?-homomorphisms fi : Pi —>¦ B{. 0 <
i < n such that.
E.7)
di+1fi+1 = fidi+1 forO<i<n-l.
Since Pn+\ is projective. the sequence Bn+i ^ Bn -4 i?re-i is exact and
the homomorphism fndn+i : Pn+i —> Bn is such that.
dn(fndn+i) = (dnfn)dn+i = (fn-idn)dn+i = fn-i(dndn+i) = 0.
it follows from Proposition 3.1.5 that there exists an i?-homomorphism
fn+i ¦ Pn+i -> Bn+1 such that. dn+ifn+i = fndn+i- This completes induc-
induction and. therefore, the existence of a chain map / = \fn} is achieved.
Next, suppose that g = {gn} is another chain map : P4 —> Br over /.
Then rjg0 = /e and dn+1gn+1 = gndn+i for n > 0.
Now rj(fo-go) = 0. Po is projective and B\ -4- Bo —> B is exact. Therefore,
there exists a homomorphism s0 : -Po —> -Bi such that 9iSo = fa — go- Take
s_i : A —> Bo to be the zero map. We thus get /o — ffo = <9iSo + s_ie.
Suppose that, n > 0 and that, we have already constructed homomor-
phisms Si : Pi ^ Bi+\ such that.
fi ~ 9i =
/o - .9o =
Consider the diagram
+ s-i
P
ra+l
/n+1 — 9n+l — Sndn+\
Since
dn+i(fn+i — 9n+i — sndn+i) = fndn+i ~ 9ndn+i ~ (dn+isn)dn+i
= (fn - 9n ~ dn+lSn)dn+i
= (sn-idn)dn+l = 0.
there exists a homomorphism sn+i : Pn+\ —>¦ P>re+2 such that
/n+l ~ 9n+l — Sndn+i = dn+2Sn+l
© 2003 by CRC Press LLC
or
fn+l ~ 9n+l = sndn+\ +
This completes induction and hence there exists a homotopy s = {sn}
between / = {/„} and g = \gn}. rj
Remark 5.1.6 Let A be an i?-module and
P : • ' • -> Pn+l ^ Pn ^ Pn-l "> • ' ¦ "> Pi ^ ^0 4 A -> 0
be two protective resolutions of A. By the comparison theorem there exist
chain maps f = {/„} : P4 -»¦ PA' and g = {gn} : I"A ->¦ P4 both over
the identity map 1a- Then gf = {gnfn} : P4 -> P4 and fg = {fngn} '¦
I"A ->¦ P^ are chain maps both over 1a- Also lp = {lpn} : P4 -»¦ P4
and lp, = {lp;} : P'4 —>¦ P'4 are chain maps over 1^. By the later part of
the comparison theorem it. follows that, gf is homotopic to lp and fg is
homotopic to lp, .
x A
5.2 Injective Resolutions
Definition 5.2.1 By an injective resolution of an i?-module A we mean
an exact sequence
TP - f| _y A 3). /?0 !L± /?1 !L± . . . __v /?re~l ^_v /?" ^4 cira+1 ,
of i?-modules and homomorphisms in which each En is an injective R-
module.
5.2.2 Given a complex
the complex
is called the deleted complex of E and is denoted by E^.
5.2.3 Given complexes
E- n \ A ^\ ZT'O \ I^l X ¦. T?n d . T7'n-\-l .
. U —7 J\ —7 Hj —7 Hj —7 ' ' ' —7 Hj —7 Hj —7 ' ' ' .
Fn \ c? ^\ z^O \ Z7I \ 1 \ zpn \ Tpn-\-l .
; u —7 -ts —7 p —7 p —7 ¦ * ' —7 p —7 p —7 ~ ' ' -
and a homomorphism / : A —> B. a family {/"} of i?-homomorphisms
fn . fin _^ pn satisfying dnfn = fn+1dn for all n > 0 and f°r) = rj\f is
called a chain map : ~Ea —^ F^ over the i?-homomorphism / : A —> B.
© 2003 by CRC Press LLC
Using the fact that, every i?-module is homomorphic image of a projective
i?-module. we proved that every module has a projective resolution. Also
every i?-module can be embedded in an injective i?-module. Using this
repeatedly we can prove (we omit the details)
Theorem 5.2.4 Every R-module has an injective resolution.
5.2.5 Examples
(a) Let R be a principal ideal domain and Q be its field of quotients.
Then both Q and Q/R are divisible i?-modules and hence are injective R-
modules (cf. Theorem 3.3.12).
Therefore
0 -> R -4 Q 4 Q/R -> 0 > 0 -> ¦ • ¦
where i is the inclusion map and tt is the natural projection, is an injective
resolution of the i?-module R.
(b) Let G = Z/pZ be the cyclic group of order p. p a prime. Z/pZ is
a subgroup of the Prufer group Z(p°°). The Prufer group is a divisible Z-
module and then so is Z(p°°)/Z/pZ. The group Z(p°°)/Z/pZ is isomorphic
to Z(p°°).
We may alternatively consider p* : Z(p°°) —> Z(p°°) given by p*(r/pk) =
r/pk~x ¦ r, k G Z, k > 1 i.e. p* is the multiplication (map) by p. The p* is
clearly an onto homomorphism with kerp* = Z/pZ. We thus get an exact
sequence
E.8) 0 -> Z/pZ -> Z(p°°) -> Z(p°°) -> 0 -> > 0 -> • • ¦
The Abelian group Z(p°°) being divisible is an injective Z-module (cf. The-
Theorem 3.3.12) and E.8) is an injective resolution of the Z-module Z/pZ.
Theorem 5.2.6 (Comparison theorem) Given a diagram
V n d° ^
X :0 A —*¦ X° >¦ >¦ Xn » Xn+1 >¦ ¦ ¦ ¦
f
E : 0 B *¦ E° *¦ *¦ En *¦ En+1 *¦ ¦ ¦ ¦
C s° sn
of R-modules and homomorphisms in which the upper row is exact and
every En in the lower complex is an injective R-module, then there exists
a chain map {/"} : X^4 —> E^ over f. Moreover, two such chain maps are
homotopic.
© 2003 by CRC Press LLC
Proof. Since E° is an injective module and rj is a monomorphism. there
exists an i?-homomorphism /° : X° —$¦ E° such that ("/ = f°V- Suppose
that n > 0 and that we have already obtained homomorphisms fl : X1 —>
Ei satisfying (f = f°r) and fi+1dl = 5l fl for alii, 0 < i < n - 1.
In the diagram
Jre — 1
-* Xn+1
5nfn
En+\
the row is exact. En+1 is an injective i?-module and
(Snfn)dn-1 = Sn(fndn-1) = Sn(Sn-1fn-1) = (SnSn-1)fn-1 = 0,
it. follows from Proposition 3.2.2. that, there exists an i?-homomorphism
jn+l . Xn+1 _^ En+1 guch that jn+l^n = finjn_ Thig completes induction
and. therefore, there exists a chain map {/"} : X4 —> Er over /.
Let {gn} : X4 ->¦ ER be another chain map over /. Take s° : X° ->¦ B
to be the zero map. Consider the diagram
d°
X1
f°-9°
E°
in which the row is exact. E° is an injective i?-module and
(/° - g°)r> = A - A = U - U = 0.
Therefore, by Proposition 3.2.2. there exists an i?-homomorphism .s1 :
X1 -> E° such that
/°-flo = s1do = s1d° + Cs0.
Suppose that n > 0 and that, we have already constructed homomorphisms
: Xm -> E-1. 0 < m < n such that.
E.9)
5i~1si
(Here it. is understood that. E~l = B).
Consider the diagram
© 2003 by CRC Press LLC
rln ~ 1 rln
—1 a ^ vn a
fn _f/n_Sn-lftn
Er-
in which the row is exact. En is an injective i?-module and
Un - gn - 8n~lsn)dn-1 = fndn~l-g71^-1-8n-l{sndn-1)
= Sn-\fn-1 - 5"" V - Sn-1(sndn-1)
= 8^-l^n-l _gn-l _sndn-l^
= Sn-1(Sn-2sn-1) (by E.9))
= 0.
Therefore there exists an i?-homomorphism sn+1 : Xn+1 —> En such that.
/" - gn - 8n-xsn = sn+1dn
or
This completes induction and. therefore, there exists a homotopy \sn} be-
between {/"} and {gn}. n
Remark 5.2.7 Let A be an i?-module and
. U —7 J\ —7 Hj —7 Hj —7 " ' ' —7 Hj —7 Hj —7 ' ' ' -
Y'0->i4 Y° —$¦ Y1 —$¦•••''—> Yn ^-> Yn+1 >
be two injective resolutions of A. By Theorem 2.6 there exist chain maps
{/"} : E,4 -»¦ Y^ and {g™} : Ya -> E4 both over the identity map 1a-
Then {gnfn} : E^ -> E^ and {/"#"} : Y^ -> Y^ are chain maps both
over 1a- However {l^™} : ~Ea —> ^a is a chain map over 1a and so is
{ly»} : Ya —> Ya- Therefore, by Theorem 2.6. the chain maps \gnfn}
and {Is™} : E/\ —> E4 are homotopic and so are the chain maps {fngn}
and{ly«}:Y4 ^YA-
5.3 Derived Functors
5.3.1 We have seen that every/^-module has a projective resolution. Sup-
Suppose for the time being that for every i?-module we have chosen exactly
one projective resolution. Let S be another ring and T : rM —T
sM. (orAb) be a covariant functor and X" : rM. —> sM (orAb) be a con-
travariant functor. Let A. B. C be left i?-modules and let.
© 2003 by CRC Press LLC
P :
Pn+1 H1 Pn
Po
K-1
0
be the chosen protective resolutions of A. i?. C respectively. For n > 0.
define
(LnT)A = Hn(TPA) = KerTdn/ImTdn+1
and
(RnT')A = Hn(T'PA) = KerT'dn+1/ImT'dn.
Observe that, while TP a is a left complex. T'P a is a right complex. Let
/ : A -»¦ /? be an i?-homomorphism. Let {/„} : P4 ->¦ P^ be a chain map
over /. Then we have chain maps {Tfn} : TPA -> TP^ over Tf : T(A) ->
T(B) and {T'fn} : T'PB -> T'P^ over T'f : T'(B) -> T'(A). These chain
maps then induce homomorphisms
and
Hn(Tf) : (LnT)A -> (
HniT'f) : {RnT')B -> {RnT')A
which are defined by
Hn(Tf)(x + ImTdn+1) = T(fn)(x) + ImTdn+l, x G KerTdn
and
Hn(T'f)(y + ImT'd'n) = T'{fn){y) + ImT'd'n, y G KerTd'n+1
If {/^} : Pa —> P^ is another chain map over /. then {/„} and {/^} are
homotopic. But then the chain maps {Tfn} and {Tf'n} are homotopic and
so are the chain maps {T'fn} and {T'f'n}. It follows from Theorem 4.3.3.
that. Hn(Tf) = Hn(Tf) and Hn(T'f) = Hn(T'f) for every n > 0. Thus
the chain maps Hn(Tf) and Hn(T'f) are independent of the choice of the
chain map {/„}. We write Hn(Tf) = (LnT)f and Hn(T'f) = (RnT')f.
By taking lpn : Pn —> Pn. the identity map for every n > 0. we get a
chain map {lpn} : Pa —> Pa over 1a '¦ A —> A and it is then clear from the
definitions of Hn(Tf) and Hn(T'f) that both Hn(TlA) and Hn(T'lA) are
identity maps i.e. (LhTIa = l(LnT)A anfi {RuT'Ia = 1(r<*t')A f°r every
n > 0.
© 2003 by CRC Press LLC
Let g : B —> C be an i?-homomorphism and \gn} : P'B —> Pq be a chain
map over g. Then {gnfn} '¦ Pa ->¦ P'c is a chain map over gf : A -»¦ R.
Let x G KerTdn and y G KerT'd'^+1. Then, by definition.
(LnT)(gf)(x + ImTdn+1) = T{gnfn){x)-
= (T(gn)T(fn))(x)
= T(gn)(T(fn)(x))
= (LnT)g(T(fn)(x) + ImTd'n+1)
= (LnT)g((LnT)f(x + ImTdn+1))
= ((LnT)g(LnT)f)(x + ImTdn+1)
which implies that
(LnT)(gf) = (LnT)g(LnT)f
and
¦ImT'd'^) = T'(gnfn)(y) + ImT'dn
= {T'Un)T'{gn)){y) + ImT'dn
= T>{fn){T>{gn){y)+ImT>dn)
= {RnT')f{T'{gn){y)+ImT'd'n))
= {{RnT')f{{RnT')g){y
which implies that
(RnT')(gf) = {RnT')f{RnT')g.
We have thus proved that.
5.3.2 For every n > 0. LnT : rM -»¦ sM (orAb) is a covariant functor
and RnT' : rM. —> sM {orAb) is a contravariant functor.
Let h : A -»¦ B be another i?-homomorphism and {hn} : Pa -> P'B be a
chain map over h. Then {/„ + hn} is a chain map : P^ -»¦ P'B over f + h
and we get. for every n > 0. homomorphisms (LnT)(f + h) : (LnT)A -»¦
(LnT)B and (RnT')(f + h) : (RnT')B -> (RnT')A. Let x G KerTdn and
2/G KerT'd'n+l. Then
IJ(/ + /i)(i + ImT(in+i)
= T(fn + hn)(x)+ImTd'n+l
= (T(fn) + T(hn))(x) + Im Td'n+1
= T(fn) (x) + T(hn) (x) + Im Td'n+l
= (T(fn)(x) + ImTd'n+1) + (T(hn)(x) + ImTd'n+l)
= (LnT)f(x + ImTdn+1) + (LnT)h(x + ImTdn+1)
= ((LnT)f + (LnT)h)(x + ImTdn+1)
© 2003 by CRC Press LLC
This implies that.
(LnT)(f + h) = (LnT)f + (LnT)h.
Again
= (T'(fn)+T'(hn))(y)+ImT'dn
= T'(fn)(y)+T'(hn)(y)+ImT'dn
= (T'(fn)(y) + ImT'dn) + (T'(hn)(y) + ImT'dn)
= (RnT') (f)(y + Im T'd'n) + {RnT>) (h) (y + Im T'd'n)
Therefore
(RnT')(f + h) = (RnT')f + (RnT')h.
We have thus proved
Theorem 5.3.3 For every n > 0. LnT : rM. —> sM (orAb) is an ad-
additive covariant functor and RnT' : rM. —> sM (orAb) is an additive
contravariant functor.
5.3.4 LnT is called the nth left derived functor of T and RnT' is called
the nth right derived functor of T'.
It is natural that we should also discuss what may then be called right
derived functors of T and left derived functors of T'. We know that
every i?-module has an injective resolution. For the present discussion let
us assume that, for every i?-module has been chosen exactly one injective
resolution. Let A. B. C be left i?-modules and let.
E^^^bKe^-^'e^ En+l -> ¦ ¦ ¦
El : 0 -> R ^ E° ^ El 4. • • • %' E? % E^+l -+¦¦.,
be the chosen injective resolutions of A. B. C respectively.
For n > 0. define
(RnT)A = Hn(TEA) = KerTdn/ImTdn-1
and
(LnT')A = Hn(T'EA) = KerT'dn-1IImT'dn.
© 2003 by CRC Press LLC
Let / : A —> B be an i?-homomorphism and {/"} : E^ ~^ ^ib be a chain
map over /. Then {Tfn} is a chain map : T(EA) -> T(E1B) over Tf :
T(A) -> T(B) and {T'fn} is a chain map : T'(ElB) -> T'(EA) over T'(f) :
T'(B) ->¦ T'(A). These chain maps then induce homomorphisms Hn(Tf) :
Hn(TEA) -> ffn(TEB) and ffn(T'/) : Hn(T'E1B) -> ^(T'E^). These
homomorphisms are independent of the choice of chain map E^ —> EiB
over / and we get well defined homomorphisms. We write (RnT)f for
Hn(Tf) and (LnT')f for Hn(T'f). We can then check that
Theorem 5.3.5 For every n > 0. i?™T : #.M —> sM (orAb) is an ad-
additive, covariant functor while LnT' : rM —> sM (orAb) is an additive
contravariant functor.
5.3.6 Suppose now that, we make a second choice (exactly one) for a pro-
projective resolution for every i?-module and a second choice (again exactly
one) for an injective resolution for every i?-module. If X is a projective
resolution (or injective resolution) of an i?-module M in the first choice, we
take X to be a projective resolution (or injective resolution) of M in the
second choice. Let LnT. RnT denote the covariant additive functors cor-
corresponding to LnT and RnT respectively for the new choice of projective
(injective) resolutions and let LnT', RnT' denote the contravariant additive
functors corresponding to the functors RnT' and LnT' respectively for the
new choice of resolutions.
Theorem 5.3.7 For every n > 0, the functors
(a) LnTandLnT; (b) RnT' and RnV;
(c) RnTandRnT; (d) LnT' and LnT';
are naturally equivalent.
Proof. Let A be an i?-module and let. P. P be the two chosen pro-
projective resolutions of A. Let {/„} : P^ -»¦ P^ and {gn} : Pa -> Pa be
chain maps both over the identity map 1a- Then \gnfn} : Pa —> Pa and
{fn9n} '¦ Pa ->¦ Pa are chain maps again over 1A. Since {\Pri} : P^ -»¦ P^
and {lp} '¦ P a —> Pa are also chain maps over 1A. the chain maps
{dnfn}; {lpn} : Pa -> Pa are homotopic and so are the chain maps
{fndn}; {lp } '-Pa —> Pa- But then the chain maps
(i) \T(9nfn)} = \T(gn)T(fn)}.,
{TAPJ} = {lT(pn)} : T(PA) -)¦ T(PA):
(n) {T(fn9n)} = {T(fn)T(gn)},
{T(lpJ} = {lT(Pn)} : T(PA) ->¦ T(PA):
(Hi) {T'(gnfn)} = {T'(fn)T'(gn)},
{T'(lpn)} = {lT'(Pn)} : T'(PA) -)¦ T>(PA)
and,
© 2003 by CRC Press LLC
\T\fn9n)} = \T>{gn)T>{fn)},
)^T{PA)
are homotopic. Since for any complex X. {lxn} : X —>¦ X induces the
identity map from Hn(X.) —$¦ Hn(X.) for every n > 0;
Hn(T(gf)) : Hn{TPA) -> Hn{TPA): Hn{T(fg)) : Hn{TPA) -> Hn{TPA):
Hn{T'{gf)) : Hn{T'PA) -> Hn(T'PA):
-W (J \J9)):H i1 Pa)^H {i Pa)-,
are all identity maps. Thus the compositions
(LnT)A Hn^f) (LnT)A Hn^9) (LnT)A
(LnT)A Hn^g) (LnT)A Hn^f) (LnT)A
{RnT')A Hn^9) (RnT')A Hn^'f) (RnT')A:
{RnT')A "n{4f) {RnT')A H"^'9) (RnT')A
are all identity maps of modules indicated. Hence Hn{Tf) : (LnT)A -»¦
(LnT)A is an isomorphism with Hn(Tg) as its inverse and Hn{T'g) :
{RnT')A -> (RnT')A is an isomorphism with Hn(T'f) as its inverse.
To prove that, the isomorphisms are functorial. let. B be another R-
module. h : A —> B be an i?-homomorphism and P'. P' be the two chosen
projective resolutions of B. Let {hn} : Pa —> P'b and {hn} : Pa —> P'b be
chain maps over the homomorphism h. Then we have chain maps \hnfn] :
PA —> P'B over hi a = h and \knhn} : PA —> P'B over l^/i = h, where of
course {kn} :P'B -> P^ is a chain map over 1b- The chain maps {hnfn}
and \knhn} being both over h are homotopic. Therefore the chain maps
{T{Kfn)} = {T(hn)T{fn)} and {T{knhn)} = {T{kn)T{hn)} over T(h)
are homotopic and so are the chain maps {T'(hnfn)} = {T'{fn)T'{hn)}
and {T'{knhn)} = {T>\hn)T\kn)}. Then the homomorphisms induced by
{T{hn)T{fn)} and {T(kn)T(hn)} on the homology modules are identical
and so are the homomorphisms induced on the homology modules by the
chain maps {T'(fn)T'(hn)} and {T'(hn)T'(kn)}. This proves that for every
n > 0. the diagrams
(LnT)A ^?lh {LnT)A
(LnT)h
(LnT)h
(LnT)B ^ (LnT)B
Hn(Tk)
© 2003 by CRC Press LLC
and
Rn(T'h)
(RnT')B i L (RnT)A
Hn(T'k) Hn(T'f)
(RnT')B (RnT')A
Rn{T'h)
are commutative which shows that the functors LnT and LnT are naturally
equivalent and so are the contravariant functors RnT' and RnT'. Parts (a)
and (b) of the theorem are thus proved.
Proof of the parts (c) and (d) is left as an exercise, rj
We have thus completed our task of proving that, up to natural equiva-
equivalence, the left derived functors and the right derived functors of a functor,
whether covariant or contravariant. are independent of the choice of pro-
jective or injective resolutions of modules. Tn view of this, we simply write
LnT for the nth left derived functor of T and write RnT for the nth
right derived functor of T.
5.3.8 Let M be a left i?-module and TV be a right i?-module. We have
seen earlier that. Hom,R(M, —) and TV ®R — are covariant. functors rM —>
Ab. — <Sir M is a covariant. functor from MR —> Ab and HomR(—,M) is
a contravariant functor from rM —> Ab. Homological algebra to a large
extent is concerned with the study of derived functors of these functors and
their properties.
Definition 5.3.9 We write
TorR(N,-) for LnT when T = N®r-:
tor*(-,M) for LnT when T=-®RM\
ExtR{-,M) for RnT when T = HomR(-,M):
extR(M,-) for RnT when T = HomR(M,-).
We also write, for any left i?-module A and right i?-module B, Tor^(N, A)
for (LnT)A, ExtR{A,M) for (RnT)A and extR(M,A) for (RnT)A in
the first, third and fourth cases above while in the second case we write
torR(B, M) for (LnT)B. There is a close connection between TorR(N, -)
and torR(-,M) and between ExtR(—,M) and extR(M,-) and we shall
study this connection later.
5.3.10 Given a short, exact sequence 0 -»¦ A' -»¦ A -»¦ A" -»¦ 0 of R-
modules. the left derived functors LnT for various n of A'. A. A" are
© 2003 by CRC Press LLC
related through a long exact sequence and so are the right derived functors
BnT for various n of A', A. A". To obtain these long exact sequences we
need the following two results each called Horseshoe lemma.
Proposition 5.3.11 Given a diat
d[
A'
0
A
a
ptt
A"
0
where the columns are projective resolutions of A', A" respectively and, the
row is exact, there exists a projective resolution P of A and, chain maps
f = {/„} : P^, -> P.4, 9 = {<?„} : Pa -> P^
over a and ft such that
0 -^ P^, 4 P.4 4 T"'A,, -+ 0
is an exact sequence of complexes.
Proof. For each n > 0, take Pn = P^eP" and define fn:P'n^Pn., gn :
Pn ->¦ P% by
fn(x') = (x',0), gn(x',x") = x", x' G P'n, x" G P'n.
Then Pn is a projective i?-module. fn, gn are i?-homomorphisms and the
sequence
0 -> P'n h Pn 9A PH -+ 0
© 2003 by CRC Press LLC
is exact. The module P^ being protective and ft being an epimorphism.
there exists a homomorphism r\ : Pq —> A such that fir) = e". Define
e:P0^ Abye(x',x") = ae'(x') + r}(x"), (x',x") G Po.
It is fairly easy to see that e is an i?-homomorphism and e/o = ae'. fit =
e'V If a G A, then there exists x" G P^ such that. P(a) = e"(x"). Then
P(a — r)(x")) = 0 and there exists an x' G Pq such that, a — r)(x") = ae'(x')
or a = ae'(x') + r)(x") = e(x',x") which proves that. e. is an epimorphism.
Let Ko, Ka, Kq be the kernel of e'. e. e" respectively and i' : K'o -»¦ Pg.
« : i^o -^ -Po: J" : -^o' ~^ Pq be inclusion maps. Restrictions of /o and go
to K'o and i^o induce homomorphisms /0 : if0 —> Ka. ~gQ : Ko —> Ko' with
/0 a monomorphism and ~gofo the zero map. Let (x',x") G Ko be in the
kernel of ~g0- Then x" = 0 and ae'(x') = 0. The homomorphism a being
one-one;_e'(V) = 0 i.e. x' G Ko and (x',x") = (x',0) = J0{x'). This proves
that Imfo 3 Ker~g0 and. so. Imf0 = Ker~g0-
Let x" G Ko'. Then j3r}{x") = 0 and there exists an x' G Pq sucn
r)(x") = ae'(x') or 0 = -ae'(x') +77@;") = e(-x',x"). Thus (-x',x") G
i^o and ~ga(—x',x") = x" which proves that. ~g0 is an epimorphism. This
completes the proof that the sequence
Ko>
is exact. Let d^ : P\ —> Kq. d1 : P" —>¦ Ko' be the epimorphisms induced
by d[. d'{ respectively so that d[ = id1. d'[ = i"d1. The module P" being
projective and ~ga being an epimorphism. there exists a homomorphism
rji : P" —> Kq such that. ~gor)i = d1. Define a map d\ : Pi —> Kq by
d d
The map d\ is an i?-homomorphism and d\f\ = /od-|. godi = d^gi. The
situation being the same as for e. d\ is an epimorphism. Take d\ = id\.
Then d\j\ = id\f\ = ifod\ = fo'id1 = fod[ and. similarly, godi = d'[g\.
Also i being the inclusion map. Imd\ = Imd\ = Ko = Kere. We have
thus proved that, the diagram
© 2003 by CRC Press LLC
h
Pi
/o
A1
A
A"
is commutative with exact rows and columns. Suppose that we have already
defined homomorphisms dj : Pj —»¦ Pj-i for 1 < j < n such that djfj =
fj-id'j. d'-gj = Qj-idj and Imdj = Ker dj-\ for 0 < j < n — 1. where it. is
understood that. d'o = e', do = e. d'o' = e". /_i = a. </_i = /?.
Let iT^, Kn, K" be the kernel of d'n, dn, d" respectively and i' : ^ ->
P'n, i : Kn ->¦ Pn and z" : if" ~^ P'n be the inclusion maps. Let fn be
the restriction of /„ to K'n and ~gn be the restriction of gn to ifn. An easy
diagram chasing shows that. fn takes values in Kn and ~gn takes values in
.KT". Also /„ is a monomorphism and ~gnfn is the zero map. A diagram
chasing as in the case of n = 0 shows that, the sequence
K'J, -> 0
is exact. Let d'
n+1
KL, d"
. pn
¦ rn+l
C" be the epimorphisms
induced by d'n+1. (i"+1 respectively so that d'n+1 = i'dn+1 and (i"+1 =
i"dn+1. Since gn is an epimorphism and P"+1 is a projective module, there
exists a homomorphism r/n+i '¦ P'n+\ —* Kn such that ~gnr]n+i = dn+1.
Define
: P
n+1
fra by
"), (x',x")?Pn+1.
As in the case of d\. it can be proved that dn+\ is an epimorphism and
Undn+i = ~dn+i9n+i,; fn^n+i = dra+i/ra+i. Take dra+1 = idn+i so that.
dra+i is homomorphism with dn+ifn+i = fnd'n+1., gndn+i = d"
Imdn+i = kerdr,. This completes induction. Hence
and
P :
Pn
+l
P0 4 A -> 0
© 2003 by CRC Press LLC
is a protective resolution of A and
0 -> P^, ^ PA -^ Pa" -> 0
with f = {/„} and g = \gn} is an exact sequence of complexes, rj
Proposition 5.3.12 Let 0 —> A\ ¦% A —)¦ Ai —> 0 be an exact sequence of
modules, and
El : 0 -> Ax ^ E° ^ El
d" dn _,„+!
2 ~^ ^2 ~~t ' ' ' :
be injective resolutions of A\. A^ respectively. Then there exists an injective
resolution E of A and chain maps f = {/"} : ~EiAi ~^ ^A-, g = \gn} '¦
Eyi —> ~E2A2 ower the homomorphisms f and g respectively such that
0 -> Ely4l 4 E.4 -I E2y42 ->¦ 0
is an exaci sequence of complexes.
Proof. For every n > 0. take _E" = E^®E^, fn : E? -> S™. ,g"
^ the maps defined by
fn(xi) = (xi,0); (?™(xi,X2) = X2; x\ G -E™, x2 G E%.
Then _B™ are injective modules. /". gn are module homomorphisms and
the sequences
—7 H/-I —r Hj —7 H/n —7 U
are all exact. Let L° = Cokerr}\. L\ = Cokerr}^. We thus have a diagram
© 2003 by CRC Press LLC
m
Vi
0
0
with exact rows and columns, where pi ,p2 are natural projections. The
module E® being injective and a being a monomorphism, there exists an R-
homomorphism A : A —> E® such that \a = rj\. Define a map r\ : A —> E°
by
r](a) = (\(a),mp(a))., a ? A.
The map r\ is a module homomorphism and r\a = f°rii: rj2/? = g°ri- If a G A
such that ri(a) = 0, then X(a) = 0. ri2/3(a) = 0. Then /3(a) = 0 and so there
exists ai G Ai such that, a = a{a\). Then 0 = X(a) = \a(ai) = rji(ai)
which implies ai = 0 and. therefore, a = a(a\) = 0. This proves that r\
is a monomorphism. Let L° = cokerrj and p : E° —> L° be the natural
projection. The maps /°. g° induce homomorphisms / : L® —»¦ L°. if :
L° -+ L°2, i.e.,
/ (xi + Imrji) = f°(xi) -
It is clear that if is an epimorphism and iff is the zero map. Let (x\, x2) G
E° such that 5°((xi,x2) + Imri) = 0. This means that x2 + Imr\2 = 0.
Then there exists an element a ? A such that x2 = ri2/3(a) and
Imrj
= /
- A(a),0) + Imrj
xi - A(a) + Imrn).
© 2003 by CRC Press LLC
Therefore Kerlf C Im f and. so. Kerlf = Imlf.
Let x-t G E° such that / (xi+/mrji) = 0 i.e. (xi, 0)+/mrj = 0. Then there
exists an a ? A such that (xi,0) = (A(a),rj2/?(a)); so that xi = A(a) and
rJ/3(a) = 0. The map 772 being a monomorphism /J(a) = 0 and. so. there
exists an a\ ? A\ such that, a = a{a\). Then x\ = \(a) = Aa(ai) = r?i(ai)
which implies that x-\ + Imrji = 0. Therefore / is a monomorphism. We
can define homomorphisms dn : En —$¦ En+1 exactly on the lines it has been
done in the last, proposition and prove that. E is an injective resolution of
A. That
0 -> EiAl -4 EA % E2A2 ->¦ 0
is an exact sequence of complexes again follows on the same lines, q
Theorem 5.3.13 Let 0 -»¦ A' A- A -»¦ ^4" ->¦ 0 &e an exaci sequence of
modules and T be a covariant functor from rM. to a category of modules
(or Ab). Then there exists a natural long exact sequence
• ¦ ¦ -> (Ln+1T)A (LT)/3 (Ln+1T)A" 4 (LnT)A' (L"-I)a (LnT)A ->¦ • • •
4 ( ()
Proof. Choose projective resolutions
P' :•¦¦-> i*+1 ^1 i^ ^ PL-i -»¦ •' • -> ^' ^ ^ ^ A' -)¦ 0
P" : > i^'+i ^' F» ^ Pn-i ->¦ >¦ Fi" ^ Fo' ^ ^" ->¦ °
of A', A" respectively arbitrarily. By Proposition 3.11. there exists a pro-
projective resolution
P : > Pn+l ^ Pn ^ Pn-1 ">¦ > Pi 4 P0 4 A -> 0
of ^4 and chain maps f = {/„} : P'A, —> Pa and g = {#„} : Pa —>¦ P'^,,
over a . /3 respectively such that
E.10) O^P^P^P^-^0
is an exact sequence of complexes. Observe that, for every n > 0. the
sequence
is split exact and the functor T being additive, the sequence
0 -> TP'n ^4" TPn ^4" TPH -> 0
© 2003 by CRC Press LLC
is again exact. Therefore, applying the functor T to the exact sequence
E.10) we again get an exact sequence
0 -> TP'A, ^? TVA ^f TPA,, -> 0
of complexes. Then for every n > 1. we have a commutative diagram
0
TP'
r?:_,
TPn+-A
¦+2
TPn,
TP
1 rn
TP" -
1 rn+2
TP"
1 rn
TP"
-*- -1- t-i
0
with exact rows. It follows from Theorem 4.2.4 that there is a natural exact
sequence
H{TP'n+2 - TP'n+1 - TP'n) -> H(TPn+2 - TPn+1 - TPn)
,;+1 - TP'n -
i.e.. a natural exact sequence
E.11) (Ln+1T)A' -)¦
(Ln+1T)A"
where 9 is the connecting homomorphism. Splicing together the natural
exact sequences E.11) for all possible n, we get a natural long exact se-
sequence
> (LnT)A -> (LnT)A" ->¦ (Ln_iT)A' ->¦ (Ln_iT)A ->¦ ¦ • ¦
Since P'n = Pn = P^ = 0 for all n < 0. (LnT)A etc. are all zero for such
an n and we get the desired natural long exact sequence, q
© 2003 by CRC Press LLC
Theorem 5.3.14 Let 0 -»¦ A' A- A ->¦ ^4" -»¦ 0 ftfi an exafii sequence of
R-modules and T be a contravariant functor from rA4 to a category of
modules (or Ab). Then there is a natural long exact sequence
All
0 -> (R°T)A" [it4m (R°T)A {nA'a {RQT)A> 4 {RlT)A
> {Rn~lT)A' 4 (RnT)A" (i?T)/3 (RnT)A {R)a (RnT)A'
4 (Rn+1T)A" -> ¦ • ¦
Proof. Choose arbitrary projective resolutions
and
of A' and A" respectively. By Proposition 3.11. there exists a projective
resolution
P : > Pn+1 rf4' Pn dA Pn-X -> > Pi 4 Po 4 ,4 -> 0
of A and chain maps f = {/„} : ~P'A, —> Pa over a and g = \gn} : Pa ~^
P'An over /3 such that.
is an exact sequence of complexes. For every n > 0.
0 -> PL -+ pn -> P'n -> 0
being a split exact sequence and T being an additive functor, applying T
to the exact sequence E.12). we get an exact sequence
0 -> TV"A,, -> TV A -> TP^, -> 0
In particular, for every n > 1. we have a commutative diagram
© 2003 by CRC Press LLC
TP"
1 rn-l
TP"
TP"
1 rn+
TP" -
1 rn+2
TPn
TP'
TP
1+1
TP
1 rn
¦+2"
TP,
n+2
in which the rows are exact. Theorem 4.2.4 then shows that there is a
natural exact sequence (also cf the definition of RnT for T contravariant)
E.13)
(RnT)A"
(RnT)A -> (RnT)A' 4 (Rn+1T)A"
(Rn+1T)A -> (Rn+1T)A'
where d is the connecting homomorphism. Since Pn. P^. P" are all zero for
n < 0. (RnT)A: (RnT)A' and (RnT)A" are all zero for such an n. Splicing
together the exact sequences E.13) for various n. we get the desired natural
long exact sequence, rj
Theorem 5.3.15 Let 0 —»¦ A\ A- A —>¦ A2 —>¦ 0 be an exact sequence of
R-modules and T be a covariant functor from rM to a category of modules
(or Ab). Then there exists a natural long exact sequence
0 -> (R°T)A1 {R°4}a (R°T)A {R°4H (R°T)A2 4 (R1T)A1 -> ¦ ¦ ¦
• • • —> (-ft 1 )A\ —f [It 1 )A —y [It I )A2 —f [It I )Ai
—$¦•¦¦
Proof. Choose arbitrary injective resolutions
EH v A >. Tp® >. Tpl >. >. TpTl >. TPn+l >.
i : u —> A\ —> Hii —> Hii —> • ¦ ¦ —> Hii —> Hi^ —?•••.
ri2 : U —r J±2 —r H/^ —r H/^ —r ' ' ' —r ±-j<2 —' -^2 — ' ' '
of A\ and of A2. It follows from Proposition 3.12 that, there exists an
injective resolution
E:0-> A^>- E° -> E1 -> > En -> En+1 -> ¦ • ¦
© 2003 by CRC Press LLC
of A and chain maps f = {/"} : Ei^ -»¦ E^ over a and g = {gn} : E^ -»¦
E2A2 over /? such that.
E.14) 0 -> Eix! -^ EA 4 E2A2 -> 0
is an exact sequence of complexes. Since for every n > 0.
0 -> ?x" -> En -> ?? -> 0
is a split exact sequence and T is a covariant functor, the sequence
0 -> TE? -> TS" -> TS^ -> 0
is again exact. Therefore, applying the functor T to the exact sequence
E.14). we get an exact sequence
0 -> TEiAl ->¦ TEA ->¦ TE2A2 ->¦ 0
of complexes. Tn particular, for every n > 1. we have a commutative
diagram
0
n-l
¦ TE?
¦TE[
ra+1
¦ TEn
. TEn+1 - TE%+1 - 0
0
. TE?+2 *TEn+2-
¦0
with exact rows. Theorem 4.2.4 then gives a natural exact sequence (also
cf. definition of RnT for T covariant)
{RnT)A1 -> (RnT)A -> (RnT)A2 4 (Rn+1T)A
E.15)
where d is the connecting homomorphism. Since E", En. E% are all zero
for n < 0. (i?"T)^i; (i?"T)A and (i?™T)^2 are all zero for such an n.
Combining together all the exact sequences E.15) for various n. we get the
desired natural long exact sequence, rj
© 2003 by CRC Press LLC
Theorem 5.3.16 Let 0 —> Ai —> A —> A% —> 0 be an exact sequence of
R-modules and, T be a contravariant functor from rM to a category of
modules (or Ab). Then there exists a natural long exact sequence
¦¦¦ -> (Ln+1T)A1 -> (LnT)A2 -> (LnT)A -> (LnT)A1 -> (Ln_1T)A2
-> > (LiT)Ai -> (L0T)^2 -> (L0T)A -> (LoT)Ai -> 0
Proof. Exercise.
Remark 5.3.17 Lei A be a projective R-module, B an injective R-module,
T a covariant functor from rM to a category of modules (or Ab) and U
a contravariant functor from rM to a category of modules (or Ab). We
can take a projective resolution P of A by taking Pq = A. e:P0^-Aas
the identity map 1a- Pn = 0 for all n > 1 and we can take an injective
resolution E of B by taking E° = B. r\ : B —»¦ E° as the identity map 1b
and En = 0 for all n > 1. It is then clear from the definition of left and
right derived functors and their being independent of the choice of projective
respectively injective resolutions of modules concerned, thai
Theorem 5.3.18 For all n > 1,
(LnT)A = 0; (RnU)A = 0 and (RnT)B = 0.
In particular, for every n > 1 and every N ? Mr. M ? rA4,
Tor*(N, A) = 0: ExtR(A, M) = 0, extR(M, B) = 0;
and
tor%(C, M) = 0
provided C is also a projective right R-module.
Theorem 5.3.19 If T is a right exact functor from rM (or Mr) to Ab
and U a covariant or contravariant left exact functor from rM to Ab, then
(a) LqT is naturally equivalent to T; and
(b) R°U is naturally equivalent to U.
Proof. Let A G RM and Pi -4- Po -4 A -»¦ 0 be the terminal part of
a projective resolution P of A. The functor T being right exact and the
contravariant functor U being left exact, we get exact sequences
41 tpq
and
I UP0 4-1 UP,.
These exact sequences induce isomorphisms
TPo/ImTd! -> T(A) and U(A) -> KerUdi-
© 2003 by CRC Press LLC
By definition {LQT)A ^ TP^flmTdx and (R°U)A ^ KerUdx. Hence
(L0T)A^T(A) and (R°U)A 9= U(A).
Let B be another (left) i?-module and / : A —> B be a homomorphism.
Let Qi —\- Qq —)¦ B —> 0 be the terminal part of a projective resolution Q
of B. Let {/„} : P4 —> Qs be a chain map over / (this exists because of
the comparison theorem). We then get commutative diagrams
*-+ TP0 e-
Tf0
TQ0
Td[ Te'
TA -
Tf
TB -
and
UB
UQl
0 » UA >¦ UPQ UPi
Ue Udi
with exa,ot rows. The commutative diagrams induce commutative diagrams
(L0T)A ^
(L0T)B s* TQo/ImTd',
TA
TB
and
UB ^ KerUd[ - (R°U)B
Uf
UA ^ KerUdi - (R°U)A
with horizontal maps in both the diagrams isomorphisms.
© 2003 by CRC Press LLC
This completes the proof that LqT is naturally equivalent to T and R°U
is naturally equivalent to U.
The case of U covariant left exact follows on similar lines.
Let M GR M and AT G Mr. Since the functors N (giR - . - (giR M
are right exact covariant. functors. HorriR(M, —) is a covariant. left exact
functor and HorriR(—, M) is left exact contravariant. functor, we have
Corollary 5.3.20 The functor
(a) Tor^(N, —) is naturally equivalent to N ®r —;
(b) tor^(—,M) is naturally equivalent to — ($rM;
(c) ExtR(—,M) is naturally equivalent to HorriR(—, M);
(d) extR(M, —) is naturally equivalent to HomR{M, —).
5.3.21 Exercises
1. Prove parts (c) and (d) of Theorem 3.7.
2. Complete the proof of Proposition 3.12.
3. For every covariant functor T. prove that (a) LqT is a right exact
covariant functor and (b) R°T is a left exact covariant functor.
4. For any contravariant functor T. prove that R°T is a left exact
contravariant. functor.
5. If G is an infinite cyclic group generated by an element, x(say). prove
that, the sequence
where T is the multiplication by x — 1 and e is the augmentation homomor-
phism. is exact.
6. Prove the relations E.6). Also use these and prove the exactness of
the complex E.5).
7. Tf T is covariant (contravariant) functor from rM to Ab and 0 —>
A45->C->0isa split exact sequence of i?-modules. prove that, the
sequence
0 -> T(A) TH} T(B) TH} T{C) -> 0
for T covariant and
0 -> T(C) TH] T{B) T^] T(A) -> 0
for T contravariant. is again split exact.
8. Complete the proof of Theorem 3.19 when U is a covariant left exact
functor.
© 2003 by CRC Press LLC
Chapter 6
Torsion and Extension
Functors
The aim of the present chapter is to study some ba,sio properties of the
extension and torsion functors. However, before we study these we need
to explore relationships (a) between TorR(A,B) and tor%(A,B): and (b)
between ExtR(A,B) and extR(A,B). It is also proved that TorR(A,—)
and TorR(—,B) commute with direct sums and direct limits.
6.1 Derived Functors-Revisited
6.1.1 Let T be a covaxiant additive functor and U be a contravaxiant.
additive functor both from rM. to a category of modules (or Ab). Let
A G RM.
P : • ¦ ¦ -> Pn+1 "^ Pn ^ Pn-1 -> • ¦ ¦ ^ P0 4 A -> 0
be a projective resolution of A and
V-.O^A^E^E1^ > En~l ^ En ^ En+1 -> • ¦ ¦
be an injective resolution of A. For n > 0. let.
Kn = Imdn+i and Ln = Imdn.
Then
• • • -> Pn+2 H2 Pn+1 H1 Kn -)¦ 0
where dn+\ is the homomorphism induced by dn+\, is a projective resolution
of Kn and
0 -> Ln 4 En+1 ^-X En+2 -> ¦ • ¦
145
© 2003 by CRC Press LLC
where i is the inclusion map. is an injective resolution of Ln. Since the
definition of derived functors is independent up to natural equivalence, of
the choice of projective resolution / injective resolution of modules.
{LYT)Kn ** KerTdn+2/ImTdn+3 9* (Ln+2T)A:
(RlT)Ln =s KerTdn+2/ImTdn+1 9* (Rn+2T)A:
(RlU)Kn 9= KerUdn+3/ImUdn+2 ^ (Rn+2U)A.
We have thus proved
Theorem 6.1.2 (a) (L^Kn ^ (Ln+2T)A, (b) (RlT)Ln Sa (Rn+2T)A
and (c) (RlU)Kn ^ (Rn+2U)A.
Corollary 6.1.3 If N G Mr and M G RM, then
(a) Tor*+2(N, A) - Tor?(N, Kn);
(b) extn+2{M,A)^extE{M,Ln)-
(c) ExtR+2(A,M) ^ ExtlR(Kn,M).
Remark 6.1.4 The results of Theorem, 1.2 are equally true if left m.odules
are replaced by right modules. In particular, if A is a right R-module,
P is a projective resolution and Kn is defined for n > 0 as before, then
(LiT)Kn = (Ln+2T)A. In particular, if M is any left R-module, then
tor%+2(A,M) ^ tor?(Kn,M). for every n > 0.
Theorem 6.1.5 For all m > 1. n > 1. M G RM and N G Mr,
\Lm+i± )J\n-i = \Liml )J^n; (,-K U J-n-n-l = (,-K <-> )^n;
and
(Rm+lT)Ln-1 ^ (RmT)Ln.
In particular
Tnr^ (N K , ^ — Tnr^lN K V KlrtrnJr^ (K , W^ — Krf™^ W^
and
Proof. Since Kn = Imdn+1 = Kerdn: and L" = /mrf" = Kerdn:
we have exact sequences
F.1) 0 -> ifn 4 Pn ^ _ftTn_! -> 0
F.2) 0 -> L™ -> En ^ L" -> 0
© 2003 by CRC Press LLC
The long exact sequences for LnT. RnU associated with the exact se-
sequence F.1) and for RnT associated with the exact sequence F.2) then
yield exact sequences
(LmT)Pn -> {L
(RmU)Pn -> (RmU)Kn -> (flm+It/)^»-i -> (Rm+lU)Pn:
(RmT)En -> (RmT)Ln -> (Rm+lT)Ln-1 -> (Rm+lT)En.
Since (LmT)Pn = (RmU)Pn = 0 and (RmT)En = 0 for all m > 1.
the above exact sequences yield the desired isomorphisms. The functors
N ®r —. Hom,R(M,—) being covariant additive and Hom(—,M) being
contravariant additive, the isomorphisms obtained for T and U become
TorR+l{N,Kn^)^TorR{N,Kn).,
Ext™+l{Kn_uM) -
and
Corollary 6.1.6 For all n > 1. M G i?A4 <mrf A^ G A4fl,
Tor*(N,K0) ~ Torf (N,^^), ExtR(K0,M) S* ExtR{Kn_uM) and
extR(M,L°) ^
Remark 6.1.7 4s in the case of covariant functor : ^.M —> Ab, ifT were
given to be. a covariant functor : Mr —> Ab, and A were a right R-module ,
we can prove that for all m. > 1. n > 1 (Lm+iT)Kn-i = (LmT)Kn and in
particular tor^+l(Kn-i,M) = tor^(Kn,M). This last isomorphism then
implies that tor^(Kn_i,M) = tor^(K0,M).
6.2 Torsion and Extension Functors
Theorem 6.2.1 Let A be a right R-module and B be a left R-module. Then
for all n > 0: tor%(A, B) ^ Tor*{A, B).
Proof. We have proved earlier (cf Corollary 5.3.20) that. TorR(A, -) is
naturally equivalent to A (Sir — and torR(—,B) is naturally equivalent to
- ®rB. Therefore
TorR(A, B)sz A®EB^ torR(A, B).
We now suppose that n > 1.
Let (P,rf) be a projective resolution of A and (Q,d') be a projective res-
resolution of B. Let Kn-i = Imdn. and Ln-Y = Imd!n for n > 1. Consider
the exact sequences
F.3) 0 -> K0A Po 4 A -> 0; 0^Lo^Qo^B^0
© 2003 by CRC Press LLC
where i, j are the inclusion maps. Since TorR(A, —) is naturally equivalent
to A®r— and torR(—, B) is naturally equivalent to — ®rB, the initial parts
of the long exact sequences for TorR(A, —) and torR(—,B) corresponding
to a short exact sequence of left or right i?-modules take the form
TorR(A,.) -> TorR(A,.) -> A ®n . -> A <gin . -> A <gin . -> 0
and
torf (., 5) -> torf (., B) ^ . ®R B ^ . ®R B ^ . ®R B ^ 0.
Consider the diagram
0
Po ® B —^ 0
J
0 0 0
(We suppress R. from <S>r. torR(-, -) and TorR(-, -) for simplification of
notation.) The first and third columns are exact sequences corresponding
to the first sequence in F.3) for the functors torR(—,Lo) and torR(—,B)
respectively, the zeros at the top resulting from the fact that torR(P0, Lo) =
torR(Po,B) = 0. the module Po being projective. The first and third rows
are exact sequences corresponding to the second sequence in F.3) for the
functors TorR(Ko, —) and TorR(A, —). The zeros on the left result because
TorR(Ko, Qo) = TorR(A, Qo) = 0. The second row and second column are
exact because of Po and Qo being flat modules.
Since — ®r — : Mr x rM -»¦ Ab is a bifunctor. the above diagram
is commutative. The maps d. d and 8. S are the respective connecting
© 2003 by CRC Press LLC
homomorphisms. Now
tori(A,L0) = Imd = Kera = Ker((l® j)a) = Ker(j3(l® j))
This follows because j3 : Ko ® Qo ->¦ Po <g> Qo and 1 <g> j : Po ® Lo ->¦ Pa <
are monomorphisms and the diagram is commutative. Also the Ker-Coker
sequence yields an exact sequence
Ker C —> Kerj —> Ccokera —> Coker C
or
0-yJm^ Po<E)Lo/Ker0^ Po<E)Qo/Im/3
and 0 and </> being epimorphisms (also taking into account that the diagram
is commutative), the above sequence becomes
which proves that, tori (A B) = Imd = Im,8 = Tori (A, B). We have
thus proved the result, for n = 1. In addition we have also proved that.
tori (A, Lq) = Tori (Kq, B). The process leading to this result when applied
to the short exact sequences
0 -> Kn -> Pn -> *:„_! -> 0
and
0 -> LTO -> Qm -> LTO_! -> 0
leads to
Then
tor«(A,B) ^ ton(if«-2,5) ^ tor!(Kn_3,L0) = ton{
¦ ¦ ¦ =s tor! (if0) ?n-3) = ton (A, Ln_2) ^ Tor! (A, Ln_2) =*
This completes the proof, rj
Remark 6.2.2 Theorem 2.1 thus removes the distinction between
tor^(A,B) andTor^(A,B). If P is a, projective resolution of A and Q is
a projective resolution of B, we may take Tor^(A,B) = Hn(VA ®r B) or
Hn(A®RQB).
Theorem 6.2.3 For every n > 0. Tor^(—, —) is a bifunctor from M.r x
rM -> Ab.
© 2003 by CRC Press LLC
Proof. Let A ? M.r and B ? rM.- Since Tor^(A,—) is a covariant.
functor : rM —> Ab. and Tor^(—,B) is a covariant functor : M.r —>¦ .4&;
we only need to prove : If A. A1 ? M.r. B. B' ? rM. and / : A —> A'. g :
B —> B' are i?-homomorphisms then the diagram
Tor*(A,B) , Tor*{A,B<)
U
u
Toi*(A',B) —^ * Tor2(A',B')
where /*. <?* are the homomorphisms induced by /. g respectively is com-
commutative. For this, let (P,d). (P',d') be projective resolutions of A. A'
respectively and let {/„} : Pa ->¦ P'a> be a chain map over / : A -»¦ A'. We
then get chain maps
{fn §§ 1} : ~Pa ®r B —> P'a' ®r B over f (g 1b-
{In <8> g} '¦ P'a' ®R B ~^ P/\' ®R B1 over \a> <S> g\
{In <S> 5} : P/\ ig_R B —>¦ P/s (gij B' ower 1^ (g 5;
These chain maps then give chain maps
{In <S> ?}{/n <g 1} : P/i <8>i? B —>¦ P'4, (gR _B' ower A^/ (g o)(/ (g 1^)
and
{/n <g l}{ln <S> 5} : Pa ®>r B ->¦ PJ4/ (g_R B' ower (/ (g 1b')(^a <8> g)
Thus we get chain maps \ln (g <?}{/„ (g 1} and {/„ (g l}{ln (g 5} from
Pa ®r B —>¦ P^, (gR B' both over the same map
Therefore these two chain maps are homotopic and. so. induce identical
homomorphisms on the homology modules of the two complexes. However,
the chain map {ln(g<?}{/n(S>l} induces the composition of homomorphisms
Tor*(A,B) 4 Tor*(A>,B)
and the chain map {/„ (g 1}{1« <S> 9} induces the composition of homomor-
homomorphisms
Tor*(A, B) 9A Tor*(A, B1) 4 Tor*(A1, B1).
Therefore the desired diagram is commutative, q
© 2003 by CRC Press LLC
Theorem 6.2.4 For all n > 0 and left R-modules A, B, ExtR(A,B) ^
extR(A,B).
Proof. Let A. B be left .R-modules. We Imve proved that, the functor
ExtR(-,B) is naturally equivalent to Hoitir(-,B) and extR(A, -) is natu-
naturally equivalent to HomR(A, -) (Corollary 5.3.20). Therefore ExtR{A, B) =
HomR(A,B) = extR(A,B). We now suppose that n > 1.
Let (P,d) be a protective resolution of A and (E, di) be an injective
resolution of B. Let Kn-Y = Imdn. Ln~1 = Kerd™. Consider the exact
sequences
F.4) 0 -> Ko 4 Po 4 A -> 0;
F.5)
where z is the inclusion map and dx is the homomorphism induced by d\.
Since the functor ExtR(—,B) is naturally equivalent to Hom,R(—,B) and
the functor extR(A, —) is naturally equivalent to Hom,R(A,—). the initial
parts of the long exact sequences for Extn(—,B) and extn(A, —) corre-
corresponding to a short exact sequence 0—> L —> M —> N —> 0 take the form
F.6) 0 -> HomR(A,L)^ HornR(A,M)^ Hornr(A,N)
-> extR(A, L) -> ext^A, M)
and
F.7) 0 -> HomR(N,B)^ HomR(M,B) ^ HomR(L,B)
-> ExtlB{N,B) -> ExtlB{M,B).
© 2003 by CRC Press LLC
Consider the diagram
0 —- Hom(A,B)
a
extl(K0,B)
0
0
F.8)
(We suppress _Rfrom Hom,R(—, —). ExtR(—, —) and extR(—, —) for simpli-
simplification of notation). The middle column in F.8) is exact as Hom,R(Po, —)
is left exact and Pq is protective while the middle row is exact because
Ho7tir(—,Eo) is left exact and Eq is injective. The first and the third rows
are the exact sequences F.7) corresponding to the short exact sequence F.4)
and ExtR(P0, B), ExtR(P0, L°) being 0 because of Po being projective. The
first and the third columns are the exact sequences F.6) corresponding to
the short, exact sequence F.5) with extR(A, E°) and extR(Ko,E°) being 0
because of E° being injective. Also Hom,R(—, —) : rM. x rM. —> Ab being
a bifunctor. every square in the diagram F.8) is commutative. Thus F.8)
is a commutative diagram with all the rows and columns exact. Since (f>. ft
are epimorphisms. ImO = Im (/?#) = Im G^) = Imj. Therefore
F.9)
ExtlR(A,L°) ^ CokerO = Cohere ^ extR(K0,B).
The commutative diagram F.8) also yields a commutative diagram
Hom(P0,B)
Ker
Hom(K0,B)
Ker 7
Extl(A,B)
Coker a
Coker
© 2003 by CRC Press LLC
with the vertical maps isomorphisms and <f> the homomorphism induced
by 4>. The lower row is the ker-coker exact sequence applied to the part
of the commutative diagram F.8) consisting of the lower two rows. The
homomorphism /? being an epimorphism. Coker ft = 0. Also Cokera =
extl(A,B). Therefore the above diagram takes the form
ib
Hom(P0,B) ——- Hom(K0,B) - Extl(A,B) - 0
Ker C - Kerj - ext1{A,B) - 0
in which both the rows are exact. Therefore
F.10) ExtR(A, B) ^ extR(A, B).
The process leading to the isomorphism F.9) when applied to the short,
exact sequences
0 -> Kn -4 Pn dA Kn-X -> 0
and
0, T rn — 1 \ rpra l. t m . r>
—t h —)¦ Hz —> h —> U
together with F.9) and F.10) gives an isomorphism
extR{Kn_uLm) - ExtR{Kn_uLm) - extlR(Kn, Lm~l) - ExtR{Kn,Lm~l)
Therefore, we get
Remark 6.2.5 In view of Theorem, 2.4 we may take
ExtR(A,B) = Hn(HomR(PA,B)) or Hn(HomR(A,EB)):
where P is a ¦protective resolution of A and E is an injective resolution of
B.
Theorem 6.2.6 For every n > 0. ExtR(—,—) : RM x RM ->¦ Ab is a
bifunctor covariant in the second variable and contravariant in the first.
Proof. Since for any A G RM., ExtR(A, -) : RM -»¦ Ab is a covariant
functor and ExtR(—,A) : RM —> Ab is a contravariant functor, we only
need to prove that :
© 2003 by CRC Press LLC
If A A', B, B' ? RM and / : A1 -> A, g : B -> B' arefl-homomorphisms.
then the diagram
ExtR(A,B)
ExtR(A,B')
/*
ExtR(A',B)
ExtR(A',B')
F.11)
is commutative. Let (/bfP,d). (P',<i') be projective resolutions of A. A'
respectively. Let {/„} : P'4, -> P^ be a chain map over the homomorphism
/. Let \ln] : Pa —> Pa or ~P'a' ~^ P'/!' ^e *ne r-hain map over \a or \a' in
which ln{x) = x for every x ? Pn (respectively x ? P^). We then get chain
maps
\Hom(fn,lB)} : HomR(PA,B) -> HomR(P'A,,B);
{Ham(fn,lB')} ¦¦ HomR(PA,B') ->¦ HomR(P'AI,B'):
{Hom(ln,g)} : HomR(PA,B) ->¦ HomR(PA,B'):
\Hom(ln,g)} : HomR(P'A,,B) ->¦ HomR(P'A,,B>)
over
Hom(f,lB) ¦¦ HomR(A,B) -> HomR(A',B),
Hom(f,lB<) ¦¦ HomR(A,B') -> HomR(A',B'),
Hom(lA,g) ¦¦ HomR(A,B) -> HomR(A,B'):
Hom(lA>,g) : HomR(A',B) -> HomR(A',B')
respectively . Then
is a chain map over Hom(lA',g)Hom(f, 1b) = Hom(f,g) and
{ffom(/n,lB0#om(ln,5)} :HomR(PA,B) ^ HomR(P'AIJB')
is a chain map over Hom(f, lB')Hom{lA,g) = Hom(f,g). Therefore the
two chain maps
{Hom(ln,g)Hom(fn,lB)} and {Hom(fn,lBi)Hom(ln,g)}
are homotopic and. so. induce identical homomorphisms on the homology
modules of the two complexes concerned. The chain map {Hom{ln,g)Hom
(fn, Is)} induces homomorphism which is the composition
ExtR(A,B) 4 ExtR(A',B) h ExtR(A',B')
© 2003 by CRC Press LLC
and the chain map {Hom(fn, lB')Hom(ln, g)} induces homomorphism which
is the composition
ExtR(A,B) h ExtR(A,B') 4 ExtR(A',B').
That the two compositions are identical implies that the diagram F.11) is
commutative, q
6.3 Some Further Properties of
6.3.1 Let Rop be the opposite ring of R. Then every left _R-module can
be made into a right i?op-module and every right i?-module becomes a left
R°P-modu\e. It is a trivial matter to check that every projective left R-
module becomes a projective right i?op-module and every projective right
.R-module becomes a projective left _Rop-module.
Proposition 6.3.2 If A is a right R-module and B is a left R-module, then
for all n > 0: Tor^(A,B) ^ TorR°P(B,A).
Proof. We have proved earlier that A®RB = B®R°PA, the isomorphism
being given by the map r : a (8 b -»¦ b (8 a. a € A. b ? B. Let P be a
projective resolution of the right -R-module A. Then P is also a projective
resolution of the left _Rop-module A. The map r gives a chain map \rn} :
Pa ?S>r B —> B 0r°p Pa in which every Tn = r is an isomorphism. This
chain map then induces an isomorphism : Hn(PA <3rB) -»¦ Hn(B<E>RoP Pa)
for every n > 0. Hence Tor^(A,B) ^ Tor^°P(B,A) for every n > 0. Q
Corollary 6.3.3 If R is a commutative ring, then Tor^(A,B) =
Tor^(B,A) for every n > 0.
Theorem 6.3.4 If R is commutative, then for all R-modules A, B and, all
n > 0. Tor^(A,B) is an R-module.
Proof. We have proved that. A <8)r B is an R-module in which the
scalar product is defined by r(a & b) = (ar &b). r ? R,a ? A. b ? B.
Since Tor§{A, B) = A®RB. we may assume that n > 1. Let (P, d) be a
projective resolution of A. For any n > 1. Pn $5 i? is an i?-module. Also
for n > 1. r ? R, x ? Pn and b? B,
(dntg)l)(r(x(gib)) = (dn(g;l)((xr)(g>b) = dn(xr)(g>b = dn(x)r<Z>b
which proves that every dn(S>l is an _R-homomorphism. Therefore Ker (dn®
1) and Im (dn+i (g) 1) are i?-submodules of Pn ®RB and then Tor^{A, B) =
Ker (dn (8 1)/Im (dn+i (8 1) is an .R-module. q
© 2003 by CRC Press LLC
Let r be an element in the centre Z(R) of R. B be a left i?-module
and [ir :/?—>/? be the multiplication map i.e. fj,r(b) = rb. b ? B. Then \ir
is an i?-homomorphism. For any right i?-module M and n > 0. fir induces
a homomorphism 1 <g) /zr : M <g)# B ^ M ®r B. where
A <g> fir)(x <g> b) = x <g> /irF) = x (8 (rt) = r(x (8) 6)
for x ? M. b ? B. Therefore 1 ® fir is also multiplication by r.
Theorem 6.3.5 Let A be a right R-module, B a left R-module, r ? Z(R)
and fir :/?—>¦/? the multiplication map. Then for every n > 0, the induced
homomorphism fj,* : Tor^(A,B) —> Tor^(A,B) is also multiplication by r.
Proof. Let P be a projective resolution of A. Then {l®fir} : Pa®rB —>¦
Pa ®r B is a chain map over 1 ® fir : A ®r B —> A ®r B. In the chain
map every morphism 1 ® /zr is multiplication by r and. therefore, the chain
map induces homomorphisms: Tor^(A,B) —$¦ Tor^(A,B) which are again
multiplication by r. rj
We can similarly have
Theorem 6.3.6 Let A be a right R-module, B a left R-module, r G Z(R)
and fj,r : A —»¦ A the multiplication map by r. Then for every n>0, the in-
induced homomorphism fi* : Tor^(A,B) —> Tor^(A,B) is also multiplication
by r.
6.3.7 Let 0—> A —> P —> B —>0bean exact sequence of right i?-modules
with P a projective module. For any left i?-module M. the long exact
sequence for TorR(—, M) corresponding to the above short exact sequence
gives
F.12) Tor*(B, M) 9* Tor*_Y (A, M) for all n > 2.
Similarly, if 0 —> C —> Q —> D —>0isan exact sequence of left i?-modules
with Q projective. then for any right i?-module /V we have
F.13) Tor*(N,D) =* Tor^N.G) for all n>2.
Theorem 6.3.8 Let {Bi}i^\ be a family of left R-modules and A be a right
R-module. Then for all n > 0,
Proof. For n = 0.
© 2003 by CRC Press LLC
For every i ? A. choose a projective module Pi and an epimorphism en :
Pi —>¦ Bi. Let Ki = Kercti. Then, we have an exact sequence
F.14) 0 -> Ki -> A ^ Bi -> 0
The family of these exact sequences then yields an exact sequence
F.15) 0^®J2Ki^®J2Pi^(BJ2Bi^°
in which © J] Pi being direct sum of projective modules is projective. Initial
part of the long exact sequence for TorR(A, —) corresponding to the exact
sequence F.15) gives an exact sequence
F.16) 0 =
Also, for every i ? A we have an exact sequence
0 = Tor*(A, Pi) -> Tor*(A, Bt) -+A®RKi^A ®r Pi
and this family of sequences leads to an exact sequence
F.17) 0 -> © Yj Torf (A> B0 ->¦ ® 1] A ®-R ^ "^ ® 1] A ®R Pi-
Since tensor product commutes with direct sums and isomorphism
M ®^2Nt = ®^2M <E) Nt is functorial. we get a commutative diagram
0 T?(AZB)
0 ZTR(AB)
with exact rows and the vertical maps are isomorphisms. This diagram then
induces a homomorphism : Tor^{A,®^Bi) —> ®^Tor^{A,Bi) making
the completed diagram commutative. An easy diagram chasing shows that
this homomorphism is an isomorphism. Thus
Tor? (A, (BY.Bi)-®^ Tori(A^ Bi)-
Suppose that n > 2 and that Tor* (A, §Y.Li) = ®Yl Tor* (A, Li) for
all m < n and for every family of left i?-modules {L{\. The long exact
sequence for TorR(A,—) corresponding to the exact sequences F.14) and
F.15) yield isomorphisms Tor%(A,Bi) = TorR_x{A,Ki) for every i and
Torn_l(A, Ki). by induction hypothesis
© 2003 by CRC Press LLC
This completes induction. [—j
Theorem 6.3.9 Let {Ai} be a family of right R-modules and B be a left
R-module. Then for all n > 0,
Proof. Using Proposition 3.2 and Theorem 3.8 we have
Proposition 6.3.10 If n > 2, Tor%(A,B) = 0 for all Abelian groups A
and B.
Proof. Let A. B be Abelian groups. Since every Abelian group is
homomorphic image of a free Abelian group, there exists an exact sequence
0—> K —> F —> A —>¦ 0 in which F is a free Abelian group. Every subgroup
of a free Abelian group being free Abelian. K is free Abelian. Thus we get
a projective resolution P of A where Pq = F. P\ = K and Pn = 0 for all
n > 2. Therefore, for n > 2. Tor%(A,B) = 0. n
We next compute Tor^(A,B) for some special rings i? and suitable
modules A and B.
Proposition 6.3.11 If R is an integral domain, r ? R. r / 0 and B is an
R-module, then Tor?(R/rR,B) ^ B[r], where. B[r] = {b G B\rb = 0}.
Proof. Since R is an integral domain and r ? R is non-zero. fir : R —»¦ i?
is a monomorphism. We then get an exact sequence
0 -> i?. ^ i?. -> i?/r.R -> 0
which is a projective resolution of R/rR with Po = P\ = R. d\ = fir.
Therefore Tor^(R/rR,B) = Ker(nr®l : R<giRB -> R®RB). As R®RB =
B and /zr §§ 1 under this isomorphism becomes /zr : B —»¦ i?. therefore.
Tor?(R/rR,B) ^ ATer (^ : B ^ B) = B[r\. n
Proposition 6.3.12 Lei R be a Euclidean domain, r, s ? R with g.c.d(r, s)
= 1 Mid 4, B ftfi R-modules with rA = 0 = sB. T/jsn Torf(A,B) = 0
A<giff 5 = 0.
© 2003 by CRC Press LLC
Proof. Let jir : A —»¦ A. /zs : B —> B be multiplication maps by r
and s respectively. Then rA = 0 = si? imply that /zr and fis are the zero
maps. Therefore [i*r : Tor?(A,B) -> Torf (A,B) and /z* : Tor?(A,B) ->
Tor^(A,_B) are also zero maps. But /x* is the multiplication by r and /z*
is multiplication by s. Therefore
rTor?(A,B) = 0 = sTorf (A, B).
Also rA = 0 = sB imply r(A <g>R B) = 0 = s(A <g)fl _B). Since r, s
are relatively coprime and i? is a Euclidean domain, there exist elements
u. v ? R such that ur+vs = 1. From this then follows that Tor^(A, B) = 0
and (A <giR B) = 0. rj
Corollary 6.3.13 W^Wi Wie hypothesis of the above proposition, if
0 —> L A Af —> R —> 0 is an exact sequence of R-modules, then
A®R L = A®RM
Proof. Terminal part of the long exact sequence for Tor corresponding
to the exact sequence 0-tLA-M-^B-tO gives an exact sequence
Tor?(A,B) -> Tor?(A,L) -> Torg(A,M) -> Tor?(A,B) -> 0.
Since Tor^(A,B) = 0, A®rB = 0 and Tor^(A, -) is naturally equivalent
to A (gifl — . the result, follows, rj
Proposition 6.3.14 Let R be a Euclidean domain, r, s G R.rs ^ 0. Then
Torf{R/rR, R/sR) ^ i?/rfi ®_r R/sR.
Proof. The terminal part of the long exact sequence for Tor(-,R/sR)
corresponding to the short exact sequence 0 —> R. —> R. —)¦ R/rR —> 0 leads
to an exact sequence
0 -> Torf{R/rR, R/sR) -> R/sR ^ R/sR -> R/rR ®R R/sR -> 0.
Therefore
R/rR <g>_R i?/si? ^ R/sR/Imfir = (R/sR)/(rR + sR)/sR
^ R/rR + sR^ R/dR,
where d = g.c.d(r, s) and
Tor^(R/rR,R/sR) = (R/sR)[r] = {a + sR\a G R and s divides ar}
Let r = rid. s = Sid so that r\. si are relatively coprime elements of R.
Therefore
Torf(R/rR, R/sR) = {a + sR\a G R and Sidivides an}
= {a + sR\a G i? and si divides a}
© 2003 by CRC Press LLC
Hence
Tor?(R/rR,R/sR) ^ R/rR®RR/sR. Q
Corollary 6.3.15 // A, B are finite Abelian groups, then Torf(A,B) =
Proof. For A, B finite cyclic, the result follows from the above propo-
proposition. Let A = & Ya=i Ai, B = © ^j=i Bj, where each one of Ai, Bj is
a finite cyclic group. Then
Torf(A, B)^®Yj Tor?(Ai> Bj)^®^Ai®BJ~A®B- ?
i,j i,j
Corollary 6.3.16 Let A, B be finitely generated Abelian groups. Then
Tori (A,B) = (tA) §§ (tB), where tX denotes the torsion subgroup of an
Abelian group X.
Proof. Since A. B are finitely generated Abelian groups.
A = A1 ®A2., B = Bi ®B2.,
where A\. B^ are torsion subgroups of A and R respectively and A2. B2
are torsion free subgroups of A. B respectively. Both A. B being finitely
generated. Ax. B\ are finite Abelian groups and A2. B2 are free Abelian
groups. Therefore
Torf(A,B) -
®Tor?(A2,B2
It is fairly easy to see that, if A is a flat right i?-module and B is a flat left
i?-module; then Tor*(A,C) = 0 and Tor*(D,B) = 0 for all n > L for all
left i?-modules C and all right i?-modules D. For the converse, we infact
have
Theorem 6.3.17 If Tor?{A, B) = 0 for all left R-modules B, then A is
flat.
Proof. Consider an exact sequence 0 —> B' —> B —> B" —> 0 of left
i?-modules. The terminal part of the long exact sequence for TorR(A, —)
yields an exact sequence
0 = Tor?(A,B") -^A®rB'^A®rB^A®rB"^0
which proves that A is flat, q
© 2003 by CRC Press LLC
6.3.18 Example
Let m = kd be an integer with d > 1. k > 1. Then
-4 Z/mZ A ZjmZ -4 iZ/mZ -> 0
is a Z/mZ-projective resolution of kZ/mZ. For any Z/mZ-module A. we
then get
Tor^mZ(kZ/mZ., A) Sa if er (A; : A -> A)/Im(d :A^A),
Tor%J%f(kZ/mZ, A) ^Ker(d:A^ A)/Im(k : A ^ A)
for all r > 0.
6.3.19 Exercises
1. If B = Z/AZ, compute Tor*(Z/2Z., Z/2Z) for n > 0.
2. If i? = Z/8Z, compute Tor%(Z/AZ, Z/2Z) for n > 0.
6.4 Tor and Direct Limits
We next prove that Tor commutes with direct limits.
Let {Aa,ir}s be a direct family of right i?-modules over a directed set
5 and B be a left i?-module. For a < j3 in S. 7rf (g: 1 : Aa <giR B ->
A$ ®r B are Z-homomorphisms and {Aa <Eir B,ir & 1} becomes a direct
family of Z-modules over S. Now lim^{Aa,7r} ®r B is a Z-module with
homomorphisms
tt« ® 1 : AQ <g)_R B
for every a ? S such that
7TQ(g:l = G173 (8 l)Grf ®1)
for every a < /J in 5. Then there exists a unique homomorphism A :
lim^{Aa <E)R B,ir <& 1} -> lim^{Aa,7r} ®R B such that \\a = ira <& 1
for every a G 5. where Xa : Aa <g>R B -»¦ lim^{Aa (gi/j _B,tt ® 1} are the
homomorphisms as in the definition of direct limit. If u ? lim^{Aa,7r} and
b ? B. there exists an a G S and aa ? Aa such that u = Tra(aa). Define a
map 0 from cartesian product
tt}xrB-
by 8(u, b) = \a(aa ® b). If also u = 7173^. choose 7 > a, ft in 5. Then
]T^f^(a ) = 7TQaa = U = 7173@") = 7T7 7Tg(fl )
© 2003 by CRC Press LLC
Therefore, there exists a S > 7 in S such that 7r*7r2(a") = 7r*7rl(a'3) or
that nsa(aa) = ?r*(a'3) . Therefore
This proves that 0 is well defined. It is fairly easy to see that 0 is bilinear
and for u G lim^{Aa,7r}. r G R, b G B, 0{ur,b) = 0(u,rb). The map 0
then induces a homomorphism
0 : \im{Aa, tt} <8>_r B —> \im{Aa ®_r B, tt §§ 1},
0(u®b) = Xa(aa®b)
where u = TTa(aa). b G B. Then
This proves that X0 = identity map on lim^{Aa,7r} ®R B. On the other
hand, if x G \im^{Aa<E)RB,TT®l} there exists an a G S" and ya G Aa®.R.B
such that x = Xa(ya). Let j/a = X]ai ® &i: where a^ G Aa. bi G -B. Then
X(x) = XXa(ya) = '^2'Ka(ai) <g) 6^. Therefore.
0X(X) = 0\2_^ Tta\O'i) ® bi) = Xa{2_^\ai ® bi)) = Xa (y ) = X.
which proves that ^A is the identity map on lim_>{Aa ®_r B, tt ® 1}. Thus
A is an isomorphism with 0 as its inverse.
We have proved the following
Proposition 6.4.1 lim^{Aa <g>R B,n ® 1} = lim^{Aa,7r} <g>R B.
We can prove the following similarly.
Proposition 6.4.2 If {Ba,ir}s is a direct family of left R-modules over a
directed set S and A is a right R-module, then
)R Ba, 1 <g) tt} = A ®
Proposition 6.4.3 Let A = lim^{Aa,7r}. There exist projective resolu-
resolutions Xa of Aa which form a direct system over S and X = lim^{X"} is
a projective resolution of A.
Proof. For every a G S. let Xq be the free i?-module with the set Aa as
a basis. For an element, a G Aa. we take xa as a basis element of Xq and let
en : Xq —> Aa be the epimorphism which on the basis elements is defined
© 2003 by CRC Press LLC
by ea{xa) = a. For a < E in S. let. A^ : Xq —)¦ Xq be the homomorphism
which on the basis elements is defined by
Then {Xg,\}s is a direct system of modules and e : {Xg, A} -> {Aa,ir}
is a morphism of direct systems. Let Xq be the free i?-module with the
set A as a basis. For a ? S. let. Xn : Xq —> Xq be the i?-homomorphism
which on the basis elements is defined by Xa(xa) = xna^,a in Aa. It
is then clear that A^A^ = \n for every a < /3. Let Y be another R-
module with homomorphism fia : Xq —> Y such that fia = /X/3A? for
eveery a < /3. Define /i : Xq —> Y by ^(x7Ta^) = ^ia(xa). a in Aa. The
map 11 is well defined and extend it to a homomorphism. For a ? Aa.
[i\a(xa) = [i(x7Ta(aj) = /ia(a;a) which implies that /zAa = /iQ for ev-
every a. That /i with this property is unique is easy to prove. Hence
Xq = lim^{X^,A}. Let e : Xq —> A be the epimorphism which on the
basis elements is defind by e(xna^) = ira(a),a ? Aa. Let Kq be the kernel
of e and let K^ be the kernel of ea. Then {Kq , A}s is also a direct system
of modules and we get a direct system of short exact sequences
where i is the inclusion map. This system of short exact sequences leads to
a short exact sequence
0 -> lim{^, A} -> lim{X^, A} -> lim{A?, A} -> 0
which on identification of lim_>.{X^, A} with Xq and of lim^{Aa,7r} with
A takes the form
0 -> lim{^, A} -> Xo 4 A -> 0.
Also, we have an exact sequence 0 —> Kq —> Xq —> A —> 0 and the two
sequences then imply that Kq = \im^.{KQ, A}. Now working with K{? and
Kq in place of Aa and A. we can find free modules X" for every a and X|
with Xi = lim^{Xf} and short exact sequences
0 -> iff -> Xf -> if^ -> 0
for every a and
0 ->#!->• Xi -> ifo -> 0.
Combining the relevant exact sequences, we get exact sequences
0 -> Kf -> Xf -> X0Q -> AQ -> 0
and
0 -> ifi -> Xi -> Xo -> A -> 0.
© 2003 by CRC Press LLC
Continuing this process, we get protective resolutions
Xa : ... -»¦ X% ->¦ X^_Y ->¦ ... -»¦ Xq ->¦ Aa ->¦ 0
of Aa for every a and
X : ... -»¦ Xn ->¦ Xn_i ->...->¦ Xo -»¦ A ->¦ 0
of t4 such that X is direct limit of the resolutions Xa. q
Theorem 6.4.4 Given a direct family \Aa,ir}s of right R-modules over a
directed set S and a left R-module B, then
Tor*(lim{Aa,ir},B) ^ \imTor*{Aa,B}
for every n > 0.
Proof. TorR(—,B) being naturally equivalent to — ®rB. the result for
n = 0 follows from Proposition 4.1. Suppose that n > 1. For every a ? S.
choose a protective resolution Xa of Aa such that \Xa, A} is a direct family
of resolutions and lim_>{Xa, A} is a protective resolution of lim_>{Aa,7r}.
Then for every a < /? in S". and every n > 1. we have A(^d^ = d^A^. Then
{Xa ®r B, A 8 1} is a direct family of complexes. Tn particular, for every
a < /3 in S and n > 1 we have a commutative diagram (we suppress i?
from A <Sir B)
This diagram induces a homomorphism
i/n(Af ® 1) : Tor*(Aa,B) -> Tor^
which is given by
From the properties of the homomorphisms A^. it follows that
is a direct family of Abelian groups. Now
TorR(lim{Aa,ir},B) ^ Hn(\im{Xa, A} ® B)
^ Hn(\im{Xa ®B,\® 1}) (Proposotion 4.1.)
For every a ? S. the commutative diagram
© 2003 by CRC Press LLC
lim_>{X^_|_1 <E> B} *- rim_>{X^ <gi B} *-\im_>{X"_l <gi B}
where /ja are the homomorphisms as in the definition of direct limit and
the maps Dn are defined by
Dn(z) = Dn{iia{u)) =
induces a homomorphism
® 1) = Ma(u) + ImDn+i, u G Kerd" (g: 1.
For a < /?. we also have 6^Hn(X^ ® 1) = 0Q. Let F be an Abelian group
with homomorphisms <j>a : Tor^(Aa,B) —> Y such that for every a < ft.
If z G lim^{X^(g)B} such that ?>„(» = 0, then there exists u G X%
such that z = fia(u)- Now 0 = Dn(j,a(u) = fia(d% & l)(u) and there exists
? > a such that /4« ® l)(u) = 0 i.e. (Ag ® 1)« ® l)(u) = 0. Therefore
? l)(u) = 0 i.e. (A? ® l)(u) G fcerd^ ® 1. Define
ImD
n+1)
1 ® 1).
0 is well defined homomorphism with <f>0a = 4>a for every a G S. That 0
with this property is unique follows easily. Hence Hn(\im^{Xa ® B}) =
\im^{Tor*(Aa,B)} and, therefore,
Tor*Qim{Aa,ir},B) = \im{Tor*(Aa,B)}. n
On similar lines we can also prove
Theorem 6.4.5 // {X^,ir}s is a direct system of left R-modules over a
directed set S and A is a right R-module, then
Tor*(A,\im{Ba,TT}) - \im{Tor*(A,Ba)}.
Next, we obtain a result in which both direct and inverse limits are
simultaneously involved.
© 2003 by CRC Press LLC
Let {Aa,i:}s be a direct system of left i?-modules over a directed set
S and R be a left i?-module. For every a < [3. n^ : Aa —$¦ A$ induces
homomorphisms
(Trfr : HomR(AP,B) -> HomR(Aa,B)
which have the property (tt")* is the identity map of HomR (Aa, B) and if
a < P < 7 in 5. then
Thus {Homn(Aa,B),ir*}s is an inverse system of Abelian groups. Let
A = \im^{Aa, tt). For every a G 5,7ra : Aa -»¦ A induces a homomorphism
,5) -> HomR(Aa,B)
such that for every a < /? in S.
Let TV be an Abelian group and tpa : N —> Homu(Aa,B) for every a ? S
be homomorphisms satisfying tpa = (tt^)*^ whenever a < j3. Let a ?
Aa. b G A$ such that ira(a) = TTfj(b). Then there exists a 7 G 5. 7 > a,/3
such that 7r2(a) = 7r^F). For any a: G TV.
This implies that 9 : N -> HomR(A,B) defined by 6»(a:)Gra(a)) = ipa(x)(a)
is well defined. The map 0 is a homomorphism and the definition shows
that -K*nQ = tpa for every a G S. Let <f> : N ^ Homn(A,B) be another
homomorphism such that ir^tp = tpa for every a G <?. For any x G TV. a G
0(a:)GrQ(a)) = <^(a:)(a) = ^a(x)(a) = KHx)(a) = 8{x)(na(a))
showing that <j)(x) = 8(x) for every x G AT. Hence <f> = 8.
This proves the following result
Proposition 6.4.6 HomR(\im^{Aa,ir}, B) ^ lim<_{HamR(Aa, B),ir*}.
6.4.7 Exercise.
Tf A is a left i?-module and (Ba,ir)s is an inverse system of left B-
modules over a quasi-ordered set S. then
Hom(A,\im{Ba, n}s) ~ \\m{HomR(A, Ba),Hom(l,ir)}s.
© 2003 by CRC Press LLC
Chapter 7
The Functor
In this chapter we study some ba,sie properties of the functor ExtR. We
prove that there is a one-to-one correspondence between ExtR(A,B) and
the set ext(A, B) of equivalence classes of extensions of A by B. Baer sum
of extensions is introduced making ext(A,B) into a group such that the
above one-to-one correspondence becomes an isomorphism of groups. It is
proved that ExtR(A, —) commutes with direct products.
7.1 Ext1 and Extensions
In this section we establish a connection between ExtR(C,A) and classes
of equivalent extensions of A by C.
Definition 7.1.1 Let A. C be left i?-modules. By an extension of A by
C we mean an exact sequence
G.1) B:0->44B^C->0
of R-modules and homomorphisms (some authors also call this an extension
of C by A). Let E(A, C) denote the set of all extensions of A by C. Two
extensions
and
E' : 0 -> A^ B' ^C -> 0
of A by C are said to be equivalent if there exists a homomorphism f :
B —»¦ B' such that fa = a' and C'f = /3. i.e. the diagram
167
© 2003 by CRC Press LLC
a C
II / II
"" a' ' /?' ' ""
is commutative. It follows from the five lemma that this homomorphism f
is an isomorphism.
7.1.2 Exercise
The relation 'equivalent extension' in the set E(A, C) of extensions of
A by C defined above is an equivalence relation.
Let ext(A, C) denote the set of all equivalence classes of extensions of
A by C into which the set E(A, C) is partitioned. Also let [E] denote the
equivalence class of extensions of A by C to which E belongs. An extension
E of A by C is called a split extension if the exact sequence E splits.
7.1.3 Exercise
If E. E' are two equivalent extensions of A by C and one of these splits,
then so does the other.
On the other hand, we also have
Lemma 7.1.4 Any two split extensions of A by C are equivalent.
Proof. Let
E:0->44bAC->0
and
E' : 0 -> A ^ B' ^ C -> 0
be two split extensions of A by C. Then there exist homomorphisms 7 :
B -> A, i : B' -> A: 0 : C -> B and 0' : C -> B' such that 7a =
lA. 7'a' = 1A,, fid = \c and /3'6' = \G<¦ Define a map / : B -> B' by
/(&) = a'7F) + 9'P(b). b ? B. The map / is an i?-homomorphism. For
any a ? A. b ? B
fa(a) = a'ja(a) + 6' [3a (a) = a'ja(a) = a'{a)
and
[3'f{b) = /3'a'7(b) + [3'6'[3{b) = [3'6'[3{b) = /?(&).
Therefore fa = a' and /?'/ = /? and the two extensions are equivalent, rj
Theorem 7.1.5 There is a one-to-one correspondence between the ele-
ments of ext(A, C) and ExfR(C, A).
© 2003 by CRC Press LLC
Proof. Let
be an extension of A by C. Choose a protective resolution
v p ff? p <H r> _fv ri v n
of C. Then there exist homomorphisms /o : Po —> B. f\ : P\
that the diagram
A such
Po
/o
G.2)
A
C
C
is commutative. The map a being a monomorphism f^di = 0 i.e. /i ?
Ker {dl : HomR{PuA) -> HomR{P2, A)). If /^ : Po -> B and /j : P: -> A
is another pair of homomorphisms such that af[ = f^d\ and /3/q = e. then
by the comparison theorem there exists a homomorphism h : Po —> A such
that /i — f[ = hd\ = d* (h) which proves that /i +Im d* = f[ +Im d* i.e. the
element /j + Ira d\ of ExtR (C, A) is independent of the choice of the pair of
homomorphisms /i : Pi —> A. /o : Po —> B satisfying af\ = fod\, Cfo = e.
Let E' : 0 -> A ^ B' ^ C -> 0 be an extension of A by C which is
equivalent to E. Then there exists a homomorphism <f> : B —± B' such that
cpa = a' and /3'(f> = /?. Let /o, /i making the diagram G.2) commutative
be given. Then the diagram
d-2
A
Po
B'
C
c
is commutative. Therefore corresponding to the extension E' we get the
same element, /j + Ira d\ of ExtlR(C, A) which corresponds to the extension
E. We therefore get a map ip : ext(A, C) -»¦ ExtRC, A) defined by
where f\. /o are the homomorphisms making the diagram G.2) commuta-
commutative.
© 2003 by CRC Press LLC
Let E' : 0 -> A ^ B' H> C -> 0 be another extension of A by C (i.e.
we are assuming the existence of E). Let go : Po —> B'. g\ : Pi —»¦ A
be homomorphisms such that a'gi = god\,[3'go = ?¦ Then ?/>([.E']) =
gx + Imd*. Thus we have a commutative diagram (with <f> and h yet to be
defined)
P -^ p0 -^- G 0
C 0
<?o II
—- p -^ p0 -1— c —- o
Suppose that ^([-B]) = ^{[E1]), i.e., /i + Imd\ = gx + Imd\. Then there
exists a homomorphism h : Po —> A such that f\ — g\ = hd\. Let b ? B.
Then there exists x ? Po such that /?(&) = e(x) = fifo{x) and b = fo(x) +
a(a). for some a ? A. Define
</>(&) = a'h(x) + go(x) + a'(a).
If we also have y ? Po. o! ? A such that, b — /o(y) + a(a'). then there exists
z ? p such that x — y = d\(z) and
fo(x-y) = a(a')-a(a)or afY(z) = fodi(z) = a(a') - a (a)
which implies that o! — a = fi(z). Therefore
a'h(y)+go(y)+a'(a')
= a'h(x) + go(x) + a'(a1) — a'hdi (z) — god\ (z)
= a'h(x) + go(x) +a'(a) +a'fl(z) - a'fY(z) +a'gl(z) - god1(z)
= a'h(x) + go{x) + a'(a).
This proves that <j> is well defined.
That 4> is an .R-homomorphism and <f>a = a', C' <f> = C follow quite easily.
This proves that E and E' are equivalent. Therefore the map ip is one-one.
To prove that tp is surjective, consider an element f+Im d\ oiExtlR(C, A)
where / ? HomR{PuA) with d*2(f) = fd2 = 0. The subset L = {{f{z),
-di{z))\z ? Pi} is a submodule of A 8 Pn. Take B = A® Po/L and define
a : A -»¦ B by
a(a) = (a, 0) + L, a ? A.
© 2003 by CRC Press LLC
The map a is an i?-homomorphism. The map : A (B Po —> C given by
(a,x) —> e(x). a ? A. x G Po, is an i?-epimorphism which vanishes on L.
This map then induces an epimorphism 0 : B —$¦ C.
0((a,x)+L) =e(x). a G A, x G Po.
Suppose that a(a) = 0 for an a G A. Then (a, 0) = (f(z), —di(z)) for some
z G P\. Therefore d\(z) = 0 which implies z = dz(t) for some t G P<i. Then
a = f(z) = fd'2(t) = 0 . as jdi = 0. Thus a is a monomorphism.
That Im a C Ker [3 is clear from the definitions of a and /?.
Let (a,x) G A 8 Po such that /?((a,:r) + L) = e(a;) = 0. Then there exists
a 2 G Pi such that x = d\ (z) and
(a,x) + L = (a,d1{z)) + L = {a,d1{z)) + {f{z),-d1{z))+L
This proves that Ker f3 C Im a and the sequence
B:0->44r4C->0
is exact. Define /o : Po —> B by
fo(x) = @,x)+L, xGPo.
The map /o is an i?-homomorphism. For z G P1; i G Po.
Thus the diagram
Pi Pi
E :0 - A
Po
/o
a
0
C
C
is commutative and. therefore. ip([E]) = / + Imd\ which proves that the
map tp is onto, rj
Remark 7.1.6 Observe that ifE-.O^A-^-BAc^-Oisa split
extension of A by C with 7 : B —> A as a splittinq homomorphism and
© 2003 by CRC Press LLC
fo : Po —> B, fi : Pi —> A are R-homomorphisms making the diagram G.2)
commutative, then
h = Wi = 7"/i = ifodi G Imd*.
Thus ip([E]) = 0 showing that under the bisection ip : ext(A, C) —>¦
ExtR(C,A), to the class of split extensions corresponds the zero element of
ExtR(C,A).
Remark 7.1.7 The one-to-one correspondence tp forces an addition (in
fact unique) in ext(A, C) which makes ext(A, C) an Abelian group with
the map tp as an isomorphism. However, we consider explicitly the way in
which a composition is defined in ext(A, C).
Proposition 7.1.8 Given an extension
of A by C and a homomorphism A : A —> A' of modules, there exists an
extension
E' : 0 -> A' ^ B' ^ C -> 0
of A' by C and a homomorphism fi : B —»¦ B' making the diagram
A
G.3) a' /?'
commutative. More over two such extensions of A' by C are equivalent.
Proof. Consider A1 ® B and let K = {(X(a),-a(a))\a G A}. Then
if is a submodule of A' ffl B and we take B' = (A' 8 B)/K. Also define
a' : A' -> B', /?' : B' -> C, fi : B -> B' by
a'(a') = (a',0) + if; a1 ? A',
P'{{a',b)+K) = P(b), a! d A', b G B:
= @,b) + K, &G 7?.
These maps are well defined homomorphisms.
Now (a',0) G K implies (a',0) = (A(a), —a(a)) for some a G A from which
it follows that a(a) = 0 and a = 0. as a is a monomorphism. Then
o! = A(a) = A@) = 0. This proves that a' is a monomorphism. It is clear
from the definitions of a'. /?' that Imcr' C Ker /3'.
© 2003 by CRC Press LLC
Let (a', b) &A' ®B such that /?'((a', b) + K) = 0. Then /?(&) = 0 and there
exists an a ? A such that b — a(a). Now
= (a' + X(a),0)+K = a'(a' + X(a)).
This proves that Ker ff C Imcr' and. so. Imcr' = Ker /3'.
For any c G C. there exists ft G B such that c = /?(&) = /?'(@, 6) + if)
proving that /?' is an epimorphism. Hence
E' : 0 -> A' ^> B' ^> C -> 0
is an extension of A' by C.
For a G A & G B,
a'X(a) = (X(a),0) + K = (X(a), -a(a)) + @,a(a)) + K = n(a(a))
and
This proves that the diagram G.3) is commutative.
Let
E" : 0 -> A' ^ B" ^ C -> 0
be another extension of A' by C and /z" :/?->¦ B" be a homomorphism
such that a" A = /z"a and /?"/z" = /?¦ Define ct : A' ffi B -> B" by
<r(a', 6) = a" (a') + /z"F). a' ei'Je B.
It is then clear that a is a homomorphism and vanishes on K and, therefore,
it induces a homomorphism a : B' —> B"'.
<j((a',b)+K) =a"{a')+n"{b), a' ? A', be R.
That the diagram
, a' , P'
II
* a"' /?"' "
is commutative is clear. Hence .E'. -E" are equivalent, rj
The extension _E' constructed above is denoted by XE.
Observe that if E\ is an extension of A by C equivalent to the extension
E then XE is equivalent to XE\.
© 2003 by CRC Press LLC
Proposition 7.1.9 Given an extension
of A by C and a homomorphism v : C —»¦ C of modules, there exists an
extension
E' : 0 -> A ^ B' ^ C -> 0
and a homomorphism v' : B' —>¦ B making the diagram
0
A
A
B'
B
C"
C
0
G.4) a C
commutative. More over two such extensions of A by C are equivalent.
Proof. Take B' = {{b, c') G B®C'\f3{b) = v(c')} and define a' : A ->
/?' :B' ->C",z/' :B' -> 7? by
a'(a) = (a(a),0): a G A: /?'(&, c') = c': F,c') G B'
and
Then
E> : 0
' ^ C" ->¦ 0
is an extension of A by C" and the diagram G.4) is commutative.
Let
E" : 0 -> A ^ B" ^ C" -^ 0
be another extension of A by C" and i/" : B" -> B be a homomorphism
making the diagram
0
A
a" 6"
.- B" -—- C
0
a P
commutative. Define a : B" -> B 0 C" by
The map cr is a module homomorphism taking values in B' and the diagram
© 2003 by CRC Press LLC
A
a
B"
P"
C
IT
A
B'
a
P'
C"
is commutative.
The extension E' constructed to make the diagram G.4) commutative is
denoted by Ev. It is clear that if E\ is an extension of A by C equivalent to
E. then EYv is equivalent to Ev. q The results of the last two propositions
can also be proved by referring to 'push out' and 'pull ba,ck; of a pair of
homomorphisms. However for the proof of Theorem 1.11 (to follow) we
need the actual constructions of push out and pull back.
7.1.10 A description of ip ([Ev]).
Let
p . y p2 % P
pQ 4 C -> 0
be a protective resolution of C. E : 0 —> A A- B —)¦ C —> 0 be an extension
of A by C and A : A -»¦ A', v : C ->¦ C be module homomorphisms. Let
/i : Pi -»¦ A, /o : Po ->¦ B be homomorphisms such that the diagram
G.5)
Pi
0
Pi
A
Po
/o
B
C
C
0
a
is commutative. Then ip([E]) = /i + Imd*. where i/j is the one-to-one
correspondence as in the proof of Theorem 1.5. Let XE be the extension
0 —> A' ^> B' —> C —> 0 so that we have a commutative diagram
A
a
X
0
A'
B
B'
C
C
0
G.6) a' /?'
Combining the diagrams G.5) and G.6) we get a commutative diagram
© 2003 by CRC Press LLC
Pi
A/i
XE :0
Po —- C
M/o II
0
which shows that tp([XE}) = A/x + Imd\ = Xtp([E}).
Next, let Ei/ be the extension E" : 0 ^ A ^i B" ^i C ^ 0 and we
have a commutative diagram
0
0
A
A
a
B"
C
0
- B - C
a p
Recall that. B" = \{b,c') & B ® C'\p{b) = v{c')}. Let
P' : >P^P^P^C ^0
be a projective resolution of C". Let {<?„} : P'c, —$¦ Pc be a chain map over
v : C —> C so that, we get a commutative diagram
P
P2
•i z
ffi
ffo
Po
c
C
0
Define hn:P^ B" by
and hi : P[ ->¦ A by /ii
and make the diagram
d'o
= /i<7i. Both /io: ^i are module homomorphisms
d\
1 . w
pi
h0
A
B"
C
C
© 2003 by CRC Press LLC
commutative showing that
ip([Ev]) =h1+Imd[*.
Theorem 7.1.11 Let
E:Q^ A^> B AC ^0
be an extension of A by C, A : A —> A' and v : C —»¦ C module homomor-
phisms. Then the extensions (\E)v and \(Ev) of A' by C are equivalent.
Proof. By definition XE is the extension 0->A'^B'->C->0 where
B' = A'®B/K,K = {(\(a),-a(a))\a?A}
and the homomorphisms a'. C' are defined by
a'(a1) = (a',0) + K, a' G A'
and
P'((a', b) + K)= p(b), (a', b) G A'® B.
Then (\E)v is an extension
0 -> A' ^ B" ^ C" ->¦ 0,
where
= {((a', 6) +X,c')|(a', b) ?A'®B and C(b) = v(c')}.
Also a", p" are the homomorphisms defined by
a"(a1) = (a'(a'), 0) = ((a',0) + K,0), a' G A':
P"(((a',b) + K,c')) =c': (a',b) ?A'(BB: r' G C.
On the other hand Ev is an extension 0—> A —> Bi —> C —> 0 where
B-\ = {F,c') G B © C"|/3F) = v(c')} and the homomorphisms 7. E are
defined by
7@) = (a(a),0), a?A; 5(b,d) = d, (b,c') G B © C".
Then \{Ev) is an extension 0 ->¦ A' ^> B2 -> C" ->¦ 0. where
B2 = (A'Q
= {(a',6i) + L|a'G A', 61 GBj
= {(a', F,c')) + L I a' G A'; 6 G B, c' G C" and /3F) = i/(c')};
© 2003 by CRC Press LLC
and the homomorphisms 7'. S' are defined by
7'(a') = (a', 0) + L = (a', @,0)) + L, a' G A':
5'((a',61) + L) = S(h) = 5(b,d) = d, a' G A', h = (b,d) gBj.
Define a map a : A' © Bi ->¦ B" by
G(a',F,c')) = ((a',6) + A',c'),a' G A',F,c') GBj.
The map a is a module homomorphism and for any a ? A.
<7(A(a), (-a(a), 0)) = ((A(a), -a(a)) + K, 0) = @,0)
i.e. a maps every element, of L onto 0 in B". Therefore a induces a
homomorphism a : B-i —> B" .
a((a',(b,d)) + L) = ((a',b) + K,d),a' G A1, (b,d) GBi.
Fora' ?A', (b,d) G Bu
/3"a((a', (b, d)) +L)= /3"((a', b) + K, d) =d = S'((a', (b, d)) + L)
which show that the diagram
,7' S> ,
a" p"
is commutative. Hence (\E)v and \(Ev) are equivalent extensions,
7.2 Baer Sum of Extensions
Consider two extensions
E' :0^A^ B' ^>C^0
of ^4 by C and let. E @ E' denote the extension
Let V:^4©^4->-^4; A:G->CfflCbe the module homomorphisms in
which V(ai,a2) = a\ + a2. ai,a2 G A and A(c) = (c,c). c G C. We then
get an extension (V(i? © E'))A of A by C.
© 2003 by CRC Press LLC
Proposition 7.2.1 If E\ is an extension of A by C equivalent to E and
E[ is an extension of A by C equivalent to E', then the extensions (V(E(B
E'))A and (V(?i © E[))A are equivalent.
Proof. Exercise.
This result, then allows us to define sum of equivalence classes of exten-
extensions of A by C as follows :
Since the extensions E®E' and E' ®E are equivalent, we have [E] + [E1] =
[E<] + [E].
Proposition 7.2.2 If E' is a split extension of A by C, then for any ex-
extension E of A by C, [E] + [E1] = [E].
Proof. Let E : 0 -> A 4 B A C -> 0 be any extension of A by C.
Without loss of generality we may take E' as the extension 0 -> 4 A
4eC4C4 0. where i(a) = (a, 0). a ? A and n(a, c) = c. a ? A, c G C.
Then V(E © E') is the extension
where B' = A © B © (A © C)jK,
K = {(V(ai,a2),-(a,i)(ai,a2),O)\ai,a2 G A}
+a2,-a(a1),-a2,0)\a1,a2 G A}:
a'(a) = (a,0,0,0)+ K, a?A and
P'((a,b,ai,c) + K) = @,ir)(b,{ai,c))
= (P(b),c), a,a1?A,b?B,c?C.
Extension (V(E © E'))A may be taken as
0-> A^i B"^ic ->0 where
B" = {(b',c)\p>(b>) = A(c)}
= \((a,b,a1,c1)+K,c)\(p(b),c1) = (c,c)}
= \((a,b,ai,c) + K,c)\p(b)=c}.,
a"(a) = (a'(a),0) = ((a,0,0,0) + K,0), a G A:
and
P"({a,b,a±,c) + K,c) = c, a,a± G A, be B. cGC
© 2003 by CRC Press LLC
with j3(b) = c.
Define a map a :/?—>¦ B" by
a(b) = ((O,b,O,p(b)) + K: p(b))., b?B.
For a € A, b ? B.
aa(a) = ((O,a(a),O, 0) + K,0)
= ((-a,a(a),0,0) + (a,0,0,0) + K
and
p"a{b) = /3"(@,6,0, /3F)) + K, p{b)) =
which prove that the diagram
a j3
II
* a"' p"' J *
is commutative. Therefore (V(i?ffi E'))A is equivalent to E and. so. [E] +
'] = [E].n
Proposition 7.2.3 Given an extension
of A by C, let E be the extension 0->44B->G-^0 where a(a) =
—a(a). a ? A. Then the extension (V(E © E))A splits.
Proof. Extension V{E e ?) is 0 -> 4 ^> B' ^> G ® C ^ 0 where
B' =A®B®B/K.
K = {(ai + H2) —ct(ai), ct(a2))|a.i, a<i ? A}: a'(a) = (a,0, 0) + K. a ? A
p'{{aMM + K) = {C{b1),C{b2))., (a,bl,b2)?A®B®B.
Then extension (V(E © E))A may be taken as
G.7)
where
B"
0^
= {{b',c)
¦A±>
?B'
ail
B"^C ->
®C\p'{b') =
© 2003 by CRC Press LLC
a"(a) = ((a,0,0) + K,0), a ? A;C"((a,b1,b2) + K,c) = c
where we also have /3(&i) = C(b2) = c.
For proving that the extension G.7) splits, we construct a splitting homo-
homomorphism 7 : C ->¦??". Let c ? C and 6 G /? such that c = /?(&). Then
(@,6,6) + K,c) G B". If 6i G B is another element, such that, c = /3(&i),
then bi — b = a(a) for some a ? A. We have
@,6i,6i)+K = @,b,b) + @,a(a),a(a)) + K
= (O,b,b) + (-a + a,a(a),a(a)) + K
= @,6, 6) + if. as (-a + a, a(a), a(a)) G K.
Therefore, we have a unique element (@, b, b) + K,c) G B".
Define 7@) = (@, b, b) + K,c), where b G B with j3(b) = c. The map 7 is a
splitting homomorphism for the sequence G.7). rj
A lengthy but. routine argument also gives the following
Proposition 7.2.4 IfE\. E^. E3 are any three extensions of A by C, then
Combining all the threads together, we have proved that. ext(A, C) is an
Abelian group. We now prove
Theorem 7.2.5 The map ip : ext(A,C) -> ExtR(C,A) defined in Theo-
Theorem 1.5 is an isomorphism.
Proof. Since the map ip has already been proved to be one-one and
onto, we only need to prove that ip is a homomorphism. Let
be a projective resolution of C. Consider two extensions
Ei : 0 -> A -> Bi -> C -> 0; E2 : 0 -> A -> B2 -> C -> 0.
Choose homomorphisms /o : -Po —> B\. f\ : Pi —> A and go : -Po —>
B2- g\ '¦ Pi —> A which make the diagrams
/o II
© 2003 by CRC Press LLC
and
Pi
0
ffi
ffo
A
B2
C
C
commutative. Then, by the definition of the map ip.
rk([Ei]) = fx + Imd*1: i;([E2}) = 9l + Imd\.
Observe that.
p © p: ¦ • ¦ -> p2 © p2 {d242) p1 © pl (dl4l] p0 © p0 ^ c © c ->¦ o
is a projective resolution of C ffl C and the diagram
(d2,d2)
Pi ffl Pi Pi ffl Pi
(A,
x ffl E2 : 0
) fee)
Po © Po -—- C®C
(/o,ffo) II
ffl B2 - C ffl C
0
is commutative. Therefore, under the map ip : ext(A ffl A, C ffi C)
Ext1R(C®C,A®A),
Then as observed in 1.10. we have
</>([V((?i ffl E2))A}) =
where A is the homomorphism : p —
any x ? Px-
p ffl P} and V is : A ffl ^4 —> A. For
which shows that. (V(/i,ffi))A = A + <7i. Hence
= (/i + Im dl) + (gx + Im d\) = ^([Ex]) +
u
© 2003 by CRC Press LLC
Corollary 7.2.6 ExtR(C, A) = 0 if and only if every extension of A by C
splits.
Proof. Suppose that ExtR{C,A) = 0. Then ext(A,C) = 0. Let E be
an extension of A by C. Then [E] G ext(A, C) = 0 so that [E] = 0 i.e. [E]
is the class of split extensions and. so. E is a split extension.
Conversely, suppose that every extension of A by C splits. Then ext(A, C)
consists of a single element and. therefore, order of ExtR(C,A) is also 1.
Hence ExtR(C,A) = 0. Q
Theorem 7.2.7 Let A, R be left R-modules, r G Z(R) and (j,r : A ->¦ A be
the multiplication map by r. Then for all n > 0, the induced homomorphism
fj,* : ExtR(A,B) -»¦ ExtR(A,B) is also multiplication by r.
Proof. Let (P,d) be a projective resolution of A. Let n > 0. Let fj,*
denote the homomorphism : HomR(Pn,B) —> HomR(Pn,B) induced by
the R-homomorphism /ir : Pn —>¦ Pn. For / G HorriR(Pn,B), x G Pn.
(Mf))(x) = (f»r)(x) = f(Hr(x)) = f(rx) = rf(x) = (rf)(x).
Therefore, //*(/) = rf i.e. fj,* is multiplication by r. Also for / G HorriR(Pn,
A), xGPn+1.
1))(x) = (r(fdn+1))(x) = ((rf)dn+1)(x)
Thus (mX+i)(/) = «+iM*)(/) which implies that mX+i = +
and {/I*} : HoniR(P_A,B) ->¦ Hom,R(P_A,B) is a chain map. The chain
map being multiplication by r in every dimension, the homomorphism in-
induced on the homology modules is also multiplication by r. Hence fj,* :
ExtR(A,B) -»¦ ExtR(A,B) is multiplication by r. rj
In a similar fashion, we can also prove
Theorem 7.2.8 Let A, R be left R-modules, r G Z(R) and fir : R -> R
be the multiplication by r. Then for all n > 0, the induced homomorphism
H* : ExtR(A,B) —> ExtR(A,B) is also multiplication by r.
Proposition 7.2.9 If r in the centre of R is not a zero divisor and A is
an R-module with rA = A, then every exact sequence of the form 0 —> A —>
B -> R/rR -> 0 splits.
Proof. Since r is not a zero divisor, multiplication by r is a monomor-
phism [ir : R -»¦ R and we get an exact sequence 0 -»¦ R ^> R -»¦ R/rR -»¦ 0.
© 2003 by CRC Press LLC
Initial part of the long exact sequence for ExAr(—,A) corresponding to the
above exact sequence gives an exact sequence
HomR{R,A) ^i HomR{R,A) -> ExtR(R/rR,A) -> 0.
Since Ho7tir(R,A) = A and fj,* is multiplication by r. the above sequence
leads to an exact sequence
A^A^ ExtR(R/rR, A) -> 0
and. therefore. ExtR(R/rR,A) = A/rA = 0. Hence every extension of A
by R/rR splits, q
Proposition 7.2.10 If R is a Euclidean domain rA = 0 = sB, where r. s ?
R are relatively coprime, then every extension of A by B splits.
Proof. Let /ir : A —»¦ A and /is : B —> B be multiplication maps by r and
s respectively. Since rA = 0 = sB, both /ir and /is are zero maps. Therefore
H$ : Ext^B^A) -> Ext^B^A) and n* : Ext^B.A) -> fe^(B,^l) are
also zero maps. But //* is multiplication by r and //* is multiplication by s.
Thus rSx^(S,^l) = 0 = sExt^B^). The elements r. s being relatively
coprime. there exist, elements u,v ? R such that, ur + vs = 1. For any
x G Ext^B^A). x = 1.x = u{rx) + v(sx) = 0. Therefore Ext^B.A) = 0
and. then, every extension of A by B splits, q
7.2.11 Exercises
1. Let A be a set. B an i?-module and a : A —> B be a one-one and onto
map. Then there exists a unique composition in A making it an i?-module
and a an isomorphism.
2. Supply the missing links in the proof of Proposition 1.9.
3. Tf E, E-\ are equivalent extensions of A by C and A : A -»¦ A', v :
C -»¦ C are module homomorphisms then the extensions XE and XEi are
equivalent and so are the extensions Ev and E\v.
4. Complete the proof of Proposition 2.1.
5. Supply the missing links in the proof of Proposition 2.2.
6. Supply the missing links in the proof of Proposition 2.3.
7. Prove Proposition 2.4.
7.3 Some Further Properties of ExtnR.
When R, is a non-commutative ring. HorriR(A, B) can not be made into an
i?-module. Check that for r G R and / G Homu(A, B), the map rf defined
by (rf)(a) = rf(a). a G A. is not an i?-homomorphism. However, for R
commutative we have the following
© 2003 by CRC Press LLC
Theorem 7.3.1 Let R be a commutative ring. Then for all R-modules A,
B and integers n > 0, ExtR(A, B) is an R-module.
Proof. For r ? R. f ? Hottir(A,B) define a map rf : A —> B by
(rf)(a) = rf(a), a ? A. That rf is additive is clear because of / being
additive. For any s ? R. a ? A.
(rf)(sa) = rf(sa) = r(sf(a)) = (rs)f(a) = (sr)f(a)
= s(rf(a)) = s(rf)(a)
which proves that rf is an i?-homomorphism. With this operation HoniR(A.
B) becomes an i?-module.
Let (P, d) be a projective resolution of A. Then, for every n > 0. HorriR
(Pn,B) is an i?-module. Let / ? HomR(Pn,B). r ? R. x ? Pn+1. Then
for the coboundary operator <i*+1 : HomR(Pn,B) —> Hom,R{Pnjri,B) in-
induced by dn+i : Pn+i —> Pn we have
(d*n+1(rf))(x) = ((rf)dn+l)(x) = (rf)dn+1(x) = rf(dn+1(x))
which implies that d*n+1(rf) = rd*n+1(f). Thus the coboundary opera-
operator d*n+l is an i?-homomorphism. Therefore. Ker <i*+1 and Imd*n are R-
submodules of HorriR(Pn,B) and, then, so is the quotient module ExtR(A, B)
= Kerd*n+l/Imd*n. Q
Remark 7.3.2 In the case of torsion functor we proved that if R is a com-
commutative ring, then for all R-modules A, B and all n > 0, Tor^(A,B) =
Tor^(B,A). In the case of extension functor, we cannot even imagine that.
For example, for an integer m > l,Homz(Z, Z/mZ) = Z/mZ ^ 0 where,
as Homz(Z/mZ,Z) = 0.
Remark 7.3.3 Let 0—> A —> E —> B —> 0 be an exact sequence of modules
in which E is injective. The long exact sequence for ExAr(M,—) for any
R-module M applied to the above exact sequence gives an isomorphism
ExtR(M,B) ^ ?^,+1(M,,4)forarin > 1.
Similarly corresponding to an exact sequence 0—> C —> P —> D —> 0 where
P is projective and any R-module N, we have
ExtnR(C,N) ^ ExtR+1(D,N)for&lln > 1.
Theorem 7.3.4 Let A be any R-module and\Bi}i^\ a family of R-modules.
Then for all n > 0,
=s HExtR(A,Bi).
© 2003 by CRC Press LLC
Proof. For n = 0.
Ext°R(A,UBi) *< HomR(A,UBi) s* HHomR(A,Bi) s* HExt°R(A,Bi).
So. suppose that n > 1. For every ie A. embed Z?j in an injective module
.Ej with Li = Ei/Bi. Then we have exact sequences
G.8) 0 -> ?j -> ?j -> Lj -> 0.
This family of exact sequences then also yields an exact sequence
G.9) 0 -> ILBi -> n^ -> ILL; -> 0
in which TLEi being direct product of injective modules is injective. Initial
part of the long exact sequence for ExtR(A, —) corresponding to exact
sequences G.8) yield exact sequences
0 -> HomR(A, Bt) -> HomR{A,Et) -> HomR(A,Lt) -> ExtR(A, Bt)
which together give an exact sequence
0 ->
Also corresponding to the exact sequence G.9). we get an exact sequence
J -> 0.
Since HoniR(Ay —) commutes with direct, products (Theorem 1.4.5) the
above two sequences give a commutative diagram
HomR(A,UEi
HHomR(A,Ei)
with the vertical maps being isomorphisms. There is then induced a ho-
momorphism : ExtR(A,HBi) —> HExtR(A,Bi) making the completed di-
diagram commutative. An easy diagram chase then shows that, this homo-
morphism is an isomorphism. Proof for n > 2 is then easy, q
Theorem 7.3.5 Let B be any R-module and {Ai}i^\. be a family of R-
m,odules. Then for all n > 0; ExtR(® Y,AuB)~ ^ExtnR{Au B).
© 2003 by CRC Press LLC
Proof. Exercise.
The following is easy to prove.
Proposition 7.3.6 If A, B are any Abelian groups, then Ext^(A,B) = 0
for all n > 2.
Proposition 7.3.7 // a central element r of R is not a zero divisor and B
is an R-module, then ExtR(R/rR,B) ^ B/rB.
Proof. Since r is not a zero divisor in R. multiplication by r gives a
monomorphism fir : R —> R. Initial part of the long exact sequence for
ExtR(-,B) for the exact sequence 0 -»¦ R. 4 R. -»¦ R/rR -»¦ 0 gives an
exact sequence
HomR(R, B) 4 HomR(R, B) -> ExtR(R/rR, B) -> ExtR(R, B).
The module R being protective. ExtR(R,B) = 0. Also HomR(R,B) ^ B
and /x* is multiplication by r. Therefore, the above sequence leads to an
exact sequence B ^ B -»¦ ExtR(R/rR, B) -»¦ 0 which gives
ExtR(R/rR,B) ^ B/Imfj,r = B/rB. D
Proposition 7.3.8 // _ff is an integral domain, Q is its field of quotients
and A is a torsion R-module, then ExtR(A,R) = HomR(A,Q/R).
Proof. Initial part of the long exact sequence for ExtR(A, —) corre-
corresponding to the exact sequence 0->R->Q->Q/R->0 gives an exact
sequence
G.10) HomR(A, Q) -> HomR(A, Q/R) -> ExtR(A, R) -> ?z^(A, Q).
The i?-module Q being an injective module. ExtR(A,Q) = 0. Let / ?
HomR(A, Q). If a ? A. there exists anO/r Gi? such that ra = 0. Then
0 = f(ra) = rf(a). Since f(a) ? Q. Q is torsion free and r / 0. we get
/(a) = 0 i.e. /(a) = 0 for every a ? A which proves that / = 0. Hence
HomR(A,Q) = 0 and the exact sequence G.10) shows that. ExtR(A,R) =
HomR(A,Q/R).n
7.3.9 Example
Let m = kd where k. d are integers each > 1. The exact sequence
• • • A ZjmZ 4 ZjmZ -> • • • 4 ZjmZ 4 Z/mZ 4 kZ/mZ -> 0
is a Z/mZ-projective resolution of Z/dZ = kZ/mZ. Therefore for any
Z/mZ-module A. we get a complex
© 2003 by CRC Press LLC
Taking homology we get
Ext2zr/mZ(Z/dZ, A) Sa Ker(d : A -> A)/Im(k : A -> A)
= {a G A|da = 0}/kA
and
Ext2^z(Z/dZ, A) ^ ifer(fc : A -> A)/Im(d : A ^ A)
= {a G A|fca = 0}/dA.
7.3.10 Exercises
1. If A 5 are finite Abelian groups, prove that Ext1z(A, B)=A®Z B.
2. Compute Extz(A,B) when A. B are finitely generated Abelian
groups.
3. For a positive integer m and any Abelian group B. prove that
Ext\{Zlmz,B) ^ B/mB.
4. Complete the proof of Theorem 3.4.
5. Prove Theorem 3.5.
6. Prove Proposition 3.6.
© 2003 by CRC Press LLC
Chapter 8
Hereditary and
Semihereditary Rings
The present chapter is devoted to study some simple properties of heridi-
tary. and semihereditary rings. Dedekind domains and Prufer rings. It is
proved that every submodule of a free module over a hereditary ring is free,
where as over a semihereditary ring every finitely generated submodule of
a free module of finite rank is free and is of finite rank. The result of Car-
tan and Eilenberg characterizing hereditary rings is also proved. Over a
Dedekind domain the concepts of divisible and injective modules coincide.
The discussion of hereditary and semihereditary rings here follows very
closely that in Rotman.
8.1 Hereditary Rings and Dedekind Domains
Definition 8.1.1 A ring R. is called left hereditary if every left ideal of
R is protective as a left _R-module.
Definition 8.1.2 An intergral domain R. is called a Dedekind domain
if every ideal of R. is projective as an -ff-module.
Let R be a principal ideal domain. If / is an ideal of R. then / = Ra
for some a ? I. Since R is an integral domain. Ra = R for a/0. i.e.. a
free and. so. projective -ff-module. Hence R is a Dedekind domain.
To consider an example of a hereditary ring which is not a Dedekind
ring we have the following
Proposition 8.1.3 Let n be a positive integer. Then Zn = Z/nZ is hered-
hereditary if and only if n is a product of distinct primes.
Proof. Any ideal of Z/nZ is of the form (mZ + nZ)/nZ for some non-
negative integer m. If m = nq + r, 0 < r < n, then mZ + nZ/nZ =
189
© 2003 by CRC Press LLC
rZ + nZ/nZ. Let d = g.c.d.(r,n). Let k,l ? Z such that, d = kr + In.
Therefore dZ/nZ C rZ + nZ/nZ and the reverse inclusion being trivial,
we have rZ + nZ/nZ = dZ/nZ. Hence every ideal of Z/nZ is of the form
dZ/nZ where d is a divisor of n.
Tf n is a product of distinct primes, then (m, d) = 1 where m = n/d
which then implies that Z/nZ = dZ/nZ (BmZ/nZ i.e. every ideal of Z/nZ
is a direct, summand of Z/nZ and hence is a projective Z/nZ'-module.
Suppose that n is not. square free. Let p be a prime such that p2 divides
n. If the ideal pZjnZ is a projective Z/nZ-modu\e. it. being homomorphic
image of Z/nZ is a direct summand of Z/nZ and there exists a positive
divisor m of n such that Z/nZ = pZ/nZ © mZ/nZ. Considered as groups
o(pZ/nZ) = n/p and o(mZ/nZ) = n/m and the direct sum decomposition
shows that, n = o(Z/nZ) = o(pZ/nZ)o(mZ/nZ) = n/p.n/m. But then
n = pm. Since p2 is a divisor of n. p divides in and mZ/nZ C pZ/nZ.
Hence pZ/nZ is not a direct sumand of Z/nZ and. so. is not a projective
Z/nZ-module, rj
We next prove a result of Kaplansky on submodules of a free module
over a left hereditary ring. The well known result that every submodule of
a free module over a principal ideal domain is free then follows as a special
ca,se.
Theorem 8.1.4 (Kaplansky) If R is left hereditary, then every submod-
submodule of a free left R-module is isomomorphic to a direct sum, of left ideals of
R and hence of projective modules.
Proof. Let F be a free left R-module with basis {xu \ k ? K}. Since
every set can be well ordered, we may suppose the index set K to be well
ordered. For each k ? K. let.
Fk = ®
so that we get an ascending chain {Fk} of submodules of F with i*o = 0
and F/.+i = Fk ® Rxk- Let A be a submodule of F. Tn view of the relation
between Fk and Fk+\- we find that, every element, a of A n -Ffc+i can be
uniquely written in the form a = b + rxk- where b ? Fk. r ? R. Then
6k : An Fk+i -> R. defind by 8k(b + rxk) = r, b ? Fk, r ? R, is an R-
homomorphism and its kernal \s AC\Fk- If Ik denotes the image of 6k. then
Ik is a left ideal of R. and so. is a projective left -ff-module. Therefore the
exact sequence
0 -> A n Fk -> A n Fk+1 % h -> 0
splits. There then exists a submodule Ck of A n Fk+\ such that.
(8.1) ADFk+1 ={AnFk)®CkandCk = Ik.
© 2003 by CRC Press LLC
Let C be a submodule of A generated by UkeiiCk- Since F =
each a ? A lies in some Fk+i. Let fj,(a) be the least k such that a G Fk+i.
If C ^ A, there exists &n a ? A such that a $ C. Therefore the subset
{/x(a) | a G A, a $ C} of the well ordered set K is non - empty and hence has
a least element j (say). Then there exists ay ? A,y $ C such that /i(y) = j
and there is no element, z ? A, z $ C with /i(z) < j. Since y ? -Fj+i H A,
y = b + c. where b ? A n Fj and c ? Cj. Now b — y — c ? A and since y $ C
but c ? C. we have b $ C. Thus b ? Fj gives a contradiction to the choice
of j. This proves that A = C.
Next suppose that c-\ + C2 + -•- + cn = 0. where Cj G Cfc;. fci < fe < ••• <
kn. Then Cj G -Ffe;+i and. so.
ci + c2 + ... + cn,_, = -cra g AnFftn_1+i nckn c(AnFkn)n ckn
which is 0 because of the direct, sum decomposition (8.1). Thus cn = 0 and
ci + C2 + ... + cre_i = 0. Repeating the above argument a finite number of
times we get ci = c-i = ... = cn = 0. Hence A = © X^fceif Cfe and every Cu
is already isomorphic to a left ideal of B. q
Corollary 8.1.5 If R is left hereditary, then every submodule of a projec-
tive left R-module is projective.
Proof. Let P be a projective left -ff-module and A be a submodule of
P. Let F be a free left -ff-module such that P is a direct summand of F.
Then A is a submodule of F. Therefore A = (B^Ck- where each Cu being
isomorphic to a left ideal of R is a projective left _R-module. Direct sum of
projective modules being projective. A is a projective left _R-module. rj
As a consequence of this result we have
Proposition 8.1.6 If R is left hereditary, then Ext^A, B) = 0 for all left
R-modules A, B and all n, > 2.
Proof. Let A, B be left _R-modules. There exists a projective left R-
module Po and an epimorphism e : Po —> A. Let Kq = Kere. Then the
sequence
0 -> Ko 4 Po 4 A -> 0.
where i is the inclusion map. is exact. Since Ko is projective. we get a
projective resolution of A by taking Px = Ko and Pn = 0 for n > 2. Then,
for n > 2.
(A, B) - HiHomRiPn-x, B) - HomR(Pn, B) - HomR(Pn+1, B))
= 0. n
On the same lines we also have
© 2003 by CRC Press LLC
Proposition 8.1.7 If R is left hereditary, A a riqht R-module and B a
left R-module, then Tor^(A, B) = 0 for all n > 2.
Definition 8.1.8 If R is a commutative ring and F is a free _ff-module.
then the cardinality of any free basis of F is called the rank of F.
It is clear from the definition that every quotient of a finitely generated
R-module is finitely generated. However a submodule of a finitely gener-
generated R-module need not be finitely generated. But submodules of finitely
generated modules over a principal ideal domain are finitely generated.
Proposition 8.1.9 Let R be a principal ideal domain.
(i) If A is a suhmodule of a free R-module F, then rank A < ra.nkF.
(ii) If B is a submodule of a finitely generated R-module A, then B is
finitely generated. Also, if A is generated by n elements, then B can be
generated by < n elements.
Proof, (i) Let F be a free -ff-module with basis {xu \ k ? K}. Then
A = © 5Zfeeif Cft: where each Cu is a submodule of Aand is isomorphic to
an ideal of R. R being a principal ideal domain, every ideal of R is of the
form Ra for some a ? R and Ra = R as -ff-modules for a/0. Therefore
every Cu — R or is 0. Hence rankA < o(K) — rankF.
(ii) Let A be a finitely generated -ff-module generated by elements
ai,O2, ...,ara. Let F be a free _R-module with a finite basis X = \xi,x2,....
xn}. The map 6 : X —> A given by 8(xi) = Oj. 1 < i < n. can then be
uniquely extended to an _R-epimorphism 6 : F -»¦ A. Let A\ = 6~1(B) =
\x ? F | 6(x) ? B}. Then Ai is a submodule of F and by (i) above, there
exist, elements yi,y2, ...,ym ? Aum<n. such that. Ax =< yi,y2,...,ym >¦
Restriction of 6 to Ax in an epimorphism : A\ -»¦ B and. so. B is generated
by #B/1), #B/2), ¦¦¦,Q{ym) i-e- ^ is generated by < m(< n) elements, q
Corollary 8.1.10 If R is a principal ideal domain, then every projective
R-module is free.
Proof. Let P be a projective R-module and let. F be a free R-module
such that. P is direct, summand of F. Then, in particular. P is a submodule
of F. By (i) of the above proposition P is a free _R-module. q
We have proved that, over an hereditary ring, submodules of projective
modules are projective. The converse of this is also true. Also there is
a characterization of hereditary rings in terms of injective modules. For
proving these results we need the following characterization of projective
and injective modules.
Proposition 8.1.11 A left R-module P is projective if and only if for every
© 2003 by CRC Press LLC
p
a
Q"
with Q an injective R-module and a an epimorphism, there exists an B-
homomorphism g : P —>¦ Q such that ag = /.
Proof. Let a diagram as in the statement with Q an injective .R-module
and a an epimorphism be given. Tf P is a projective module, then there
exists an i?-homomorphism g : P —»¦ Q such that, ag = f.
Conversely, suppose that whenever a diagram as in the statement of the
proposition with Q injective and a an epimorphism is given, then there
exists a homomorphism g : P —»¦ Q such that ag = f. Consider a diagram
P
f
a
A"
of i?-modules and homomorphisms with a an epimorphism. Let A' be the
kernel of a and i : A' —> A be the inclusion map. Since every module can
be embeded in an injective module, let. Q be an injective -R-module and
a : A —> Q be a monomorphism. Let Q" = Coker(ai) and n : Q —> Q" be
the natural projection. Then we get a commutative diagram
A1
A1
A
a
A"
Q"
with exact rows. Let p : A" -»¦ Q" be a homomorphism such that pa = ira.
Since rr is a monomorphism. p is also a monomorphism. By the given
hypothesis, there exists a homomorphism /3 : P —>¦ Q such that. tt/J = pf.
© 2003 by CRC Press LLC
Let x G P. Let a ? A such that. a(a) = f(x). Therefore ¦na(a) =
pa(a) = pf(x) = ir/3(x) or C(x)—a(a) ? Kern, and, there exists an element
a' ? A' such that C(x) — a (a) = ai(a') or C(x) = a(a + i(a')). Thus, for
every x ? P. there exists an element ax ? A such that C(x) = cr(ax) and
a being a monomorphism the element. ax is unique. Define g : P —> A by
g(x) = ax. where ax is the unique element, with C(x) = cr(ax). Let x,y ? P
and r,s ? B. Choose ax,ay ? A such that /3(x) = <r(ax). /3(y) = <j{ay)-
Then
<y{arx+sy) = P(rx + sy) = r/3(x) +
= ra(ax) + sa(ay) = a(rax + say)
which implies that.
arx+sy = rax + say or g(rx + sy) = rg(x) + sg(y).
Hence g is an _R-homomorphism. Again, for x ? P
pag(x) = pa(ax) = Trcr(ax) = ttC(x) = pf(x)
and. since, p is a monomorphism. ag(x) = f(x). Hence ag = f and it.
follows that. P is a protective R-module. q
Proposition 8.1.12 A left R-module Q is injective if and only if for every
P>
Q
(8.2)
with P projective and a a monomorphism, there exists an R-homo-
morphism q : P —>¦ Q such that ga= f.
Proof. If Q is an injective i?module, then for every diagram (8.2) with a
a monomorphism and P any -ff-module. there exists an _ff-homomorphism
g : P -»¦ Q such that ga = f.
Conversely, suppose that, given a diagram (8.2) with P projective and
a a monomorphism there exists a homomorphism g : P —»¦ Q such that.
ga = f. Consider a diagram
Q
© 2003 by CRC Press LLC
of _ff-modules and homomorphisms with a a monomorphism. Let A" =
Cokera and n : A —> A" be the natural projection. Since every module
is homomorphic image of a projective R-module. let P be a projective
i?-module and a : P —> A be an epimorphism. Let P' = Ker(ira) and
i : P1 —> P be the inclusion map. Then we have a commutative diagram
a
Q
with exact rows. There then exists a homomorphism p : P' —»¦ A' such that
ap = rri. Since a is an epimorphism. p is an epimorphism. By the given
hypothesis, there exists a homomorphism /3 : P —»¦ Q such that. /3i = fp.
Let x ? P such that. cr(x) = 0. Then 0 = ira(x) which implies that.
x G P' and. then. ap(x) = ai(x) = 0. Therefore p(x) = 0. This proves that
Kera C Kerp.
Let a ? A and choose an x ? P such that <j{x) = a. If y ? P is another
element, with a{y) = a. then y — x ? Kera C Kerp so that. fp(y — x) = 0.
or fii(y - x) = 0 i.e. /?(z) = fi(y). Define 5 : A -> Q by </(a) = /3(x).
where x ? P with cr(x) = a. The map 5 is well defined and is an R-
homomorphism. Let a' ? A' and x ? P such that a(a') = a(x). Then
ira(x) = 0 so that x ? P' and a(a') = cr(x) = cri(x) = ap(x). Therefore
a! = p(x) and ga(a') = C(x) = Ci(x) = fp(x) = f(a'). This proves that.
ga = f and Q is an injective -ff-module. q
The following result of Cartan and Eilenberg characterizes hereditary
rings in terms of projective and injective modules.
Theorem 8.1.13 The following conditions are equivalent for a ring R:
(i) R is left hereditary.
(ii) Every submodule of a projective left R-module is projective.
(Hi) Every quotient of an injective left R-module is injective.
Proof. We have already proved (Corollary 1.5) that (i) implies (ii).
Suppose that (ii) holds. Now R is a free left -ff-module and every left ideal
of R. being a submodule of the projective -ff-module is projective. This
proves (i).
Next suppose that, (ii) holds. Consider the diagram
© 2003 by CRC Press LLC
Q—gL* Q" 0
(8.3)
with exact rows. Q an injective i?-module and P a projective i?-module.
By our assumption P' is also a projective module, so that there exists an
i?-homomorphism g : P' —»¦ Q such that ag = /. The module Q being
injective. there exists an i?-homomorphism h : P —»¦ Q such that, h/3 = g.
Then ah : P —> Q" is an i?-homomorphism and (ah)ft = a(h0) = ag = f.
It follows from Proposition A.12) that Q" is injective. This proves (iii).
Finally, suppose that (iii) holds. Consider the diagram (8.3) above
with exact rows. P projective and Q injective. By our assumption Q"
is injective. Therefore, there exists a homomorphism g : P —»¦ Q" such
that, g/3 = /. Then P being projective. there exists a homomorphism
h : P —»¦ Q such that, ah = g. Thus h/3 : P' —> Q is an i?-homomorphism
and a(h/3) = (ah)ft = gj3 = f. Proposition 1.11 then shows that P' is
projective. This proves that (iii) implies (ii). rj
Let R be a ring which is not left hereditary. Then there exists an
injective i?-module E with a quotient module E' which is not injective.
Since every injective module is divisible and every quotient of a divisible
module is divisible. E' is divisible but not injective. Recall that the ring
Z/p2Z. p a prime, is not hereditary (Proposition 1.3).
8.2 Invertible Ideals and Dedekind Rings
Dedekind domains in commutative algebra and in algebraic number the-
theory are introduced through what are called invertible ideals. We now
explore this connection of Dedekind domains.
Definition 8.2.1 Let R. be an integral domain and Q be its field of quo-
quotients. An ideal T of R. is called inversible or invertible if there exist
elements ai,a2,-,an G /. quq2,...,qn G Q with
(i) qJ Q R-, for every i, 1 < i < n:
(ii) E"=i Qiai = !•
For example, if a G R. a/ 0. then for the principal ideal T = Ra. we
may take a\ = a,qi = I/a and the ideal / is invertible. In particular, every
non-zero ideal in a principal ideal domain is invertible.
Let R be an integral domain with quotient field Q. Let, / be an invertible
ideal of R with Oj G /, ft G Q, 1 < i < n. satisfying conditions (i) and (ii)
© 2003 by CRC Press LLC
in the definition. If a ? I. then a = X]"=i riai- where for every i, 1 < i <
n, Ti = Qia ? R. This proves that T is finitely generated.
Theorem 8.2.2 A non-zero ideal I in an integral domain R is protective
if and only if it is invertible.
Proof. Let / be protective. By the protective basis theorem (Theorem
3.1.14). there exist elements ak ? I,k ? K and i?-homomorphisms <fik :
I —> R for every k ? K such that
(a) if a ? I. then (j)k(a) = 0 for almost all k ? K:
(b) if a ? I. then a = Y,keK 4>k(a)ak-
Observe that in view of (a), the summation in (b) is finite. If b. b' ?
I,W + 0; then b<j)k{b') = 4>k(W) = 4>k(b'b) = b'<pk(b) showing that the
element qk = 4>k(b)/b ? Q is independent of the choice of b ? I, b ^ 0.
Also in view of (a) above, there are only a finite number of qk ^ 0. We
consider these qks and the corresponding o/.'s. If a ? /, a ^ 0. then qka =
(<pk(a)/a)a = 4>k{a) ? R. while if a = 0. then qka ? R. trivially. Therefore
qkl C R. Again, if a ? /, a ^ 0. then a = ^2k <j)k(a)ak = ^2k qkaak which
implies that. ~^2k qkak = 1. This proves that. / is invertible.
Conversely, suppose that. / is invertible. Let 01,02,...,on G /, and
Qi,Q2, ¦¦¦,Qn G Q be elements satisfying conditions (i) and (ii) in the defi-
definition. For k, 1 < k < n. define <f>k : I ->¦ R by 4>k(a) = aqk,a ? I. Since
Qkl Q R-, o-Qk does indeed belong to R. The <fik are i?-homomorphisms and
are finite in number. Also for any a ? I.
4k {)k ^2qkk a^2 Qkdk = o.l = a
k=i k k
Hence T is projective. rj
Corollary 8.2.3 An integral domain R is a De.de.kind domain if and only
if every non-zero ideal of R is invertible.
Exercise 8.2.4 Consider the integral domain R = {a+ i/—5b \ a,b ? Z}.
Prove that the ideal of R generated by 2 and 1 + y/—5 is not a principal
ideal. Also check that R is a Dedekind domain.
We have proved that every injective module is divisible where as there
exist, divisible modules which are not injective. However, we can have the
following
Theorem 8.2.5 An integral domain R. is a Dedekind ring if and only if
every divisible R-module is injective.
Proof. Suppose that, every divisible i?-module is injective. Let E be an
injective module. Then E is divisible and. therefore, every quotient module
© 2003 by CRC Press LLC
of E is divisible and hence injective. It follows from the theorem of Cartan
- Eilenberg (Theorem 1.13) that R. is left hereditary and. so. a Dedekind
domain.
Conversely, suppose that. R is a Dedekind domain. Let D be a di-
divisible i?-module. Let / be a non-zero ideal of R and /:/—>¦ D be
an i?-homomorphism. The ideal / is invertible. Let 01,02,...,on G /,
Qi, Q2, —, Qn G Q; where Q is the field of quotients of R. such that qj C i?
for every i. 1 < i < n and X]"=i Qiai = 1- F°r every z, 1 < i < n; let dj € D
such that/(oj) = Ojdj. Such dj exist because D is divisible. Tf a G I.
then a = (Y^qia^a = Y,{<Ha)ai and /(«) = I{Y,{<Ha)ai) = Y,{<lia)Iiai) =
J^2(qia)(aidi) = (^(g'jO^d^a = da. where d — J](ajaj)dj G -D as g^Oj G i?.
Define a map g : R. —> D by g(r) = rd. r ? R. The map g is an R-
homomorphism and extends /. Baer's criterion (Theorem 3.3.5) then shows
that D is an injective i?-module. rj
Theorem 8.2.6 Let R be, a Dedekind domain and A be a torsion-free R-
modnle. Then. ExtlR{A,B) is divisible for every R-module B.
Proof. Embed A in an injective module E. Let tE = \x ? E \ rx = 0
for some 0/r G R} be the torsion module of E. Then E/tE is a torsion-
free i?-module. Let i : A —> E be the embedding i.e. a monomorphism and
p : E —> E/tE be the natural projection. The module A being torsion-free,
the composition pi : A —»¦ E/tE is a monomorphism. Thus, we may assume
that. E itself is torsion free. There exists an exact sequence
0->44E->I->0
where E is a torsion free, divisible i?-module. Let B be any i?-module.
Initial part of the long exact sequence for Extfi(-,B) corresponding to the
exact sequence above gives an exact sequence
ExtlB{E,B) -> ExtlB{A,B) -> Ext%(X,B).
It follows from Proposition 1.6 that, the last, term of this sequence is 0.
Therefore ExtlR(A,B) is homomorphic image of ExtlR(E,B). Let r G
R,r ^ 0 and /xr : E —> E be the multiplication map. The module E
being torsion-free divisible. /xr is an isomorphism. Then so is the induced
homomorphism fj,* : ExtlR(E,B) —> ExtlR{E,B). Also fj,* is multiplication
by r (Theorem 7.2.7). Therefore ExtR(E, B) is torsion free divisible. Hence
ExtR(A,B) is divisible, q
This result enables us to prove
Theorem 8.2.7 Let R be a Dedekind domain, B be an R-module and tB
be the torsion snbm.odule of B. If there exists a non-zero elem.ent r G R with
r(tB) = 0, then tB is a direct summand of B.
© 2003 by CRC Press LLC
Proof. The module B/tB is torsion-free. Therefore ExtlR(B/tB,tB) is
divisible. Let r G R, r ^ 0. with r(tS) = 0. Then rExtlR(B/tB,tB) = 0
(Theorem 7.2.8). Therefore ExtlR(B/tB,tB) = 0. Initial part of the long
exact sequence for Extn(—,tB) corresponding to the exact sequence
(8.4) 0 -> tB -> B -> S/iS -> 0
yields an exact sequence.
0 -> HomR(B/tB,tB) -> HomR(B,tB) -> HomR(tB,tB) -> 0
which, in particular, implies that, the sequence (8.4) splits, rj
Corollary 8.2.8 Let R be a Dedekind ring, B an R-module the torsion
submodule tB of which is finitely generated. Then tB is a direct ssummand
ofB.
Proof. Exercise.
Converse of Theorem B.6) is also true. However, we shall prove it in
the next chapter after we have discussed the Universal coefficient theorem.
As another application of Theorem 2.5. we obtain a characterization of
injective modules over a Dedekind domain. If R is an integral domain with
Q as its field of quotients, then Q is torsion-free, divisible i?-module and
then HomR(Q,A) becomes a divisible i?-module for every i?-module A.
Proposition 8.2.9 Let R be a Dedekind ring, Q the field of quotients of R
and K = Q/R. An R-module A is injective if and only if ExtlR(K,A) = 0.
Proof. Tf A is an injective module, then ExtR(B,A) = 0 for every
R-module B.
Next suppose that A is an R-module for which ExtlR(K, A) = 0. Initial
part of the long exact sequence for Extn(—, A) corresponding to the exact
sequence 0—> R —> Q —> K —> 0 gives an exact sequence
HomR(Q, A) -> HomR(R, A) -> ExtlR{K, A) = 0.
Therefore A = Hom,R(R,A) (Theorem 1.4.4) is homomorphic image of
Hoitir(Q, A) which is a divisible R-module. Therefore A is divisible and
hence injective. rj
Proposition 8.2.10 Let R be a Dedekind domain and A be an R-module.
The module A is projective if and only if ExtR(A,F) = 0 for every free
R-module F.
© 2003 by CRC Press LLC
Proof. If A is protective then ExtR(A,B) = 0 for every i?-module B.
Now suppose that. ExtlR(A,F) = 0 for every free i?-module F. Let
a : B —> C be an epimorphism of i?-modules and / : A —> C be an
i?-homomorphism. Choose a free i?-module F and an epimorphism n :
F —> B. Then an : F —> C is an epimorphism. Let K = Ker(an). The
long exact sequence for ExtR(A, —) corresponding to the exact sequence
0—> K —> F —> C —> 0 gives an exact sequence
(8.5) HomR(A,F) ("S HomR(A, C) -> ExtR(A, K).
The module K being submodule of a free module is projective. Therefore
if is a direct, summand of a free module F\. Let F\ = K (B B'. Then
0 = ExtR(A,K e B') ^ ExtR(A,K) e ExtR(A,B') which implies that.
ExtR(A,K) = 0. The exact sequence (8.5) then shows that (an)* is an
epimorphism and there exists a homomorphism g : A —> F such that / =
(an)*(g) = ang = a(ng). This completes the proof that A is projective. rj
8.3 Semihereditary and Priifer Rings
Definition 8.3.1 A ring R is called left semihereditary if every finitely
generated left ideal of i? is projective. An integral domain i? which is
semihereditary is called a Priifer ring.
Observe that every left hereditary ring is left semihereditary and every
Dedekind domain is a Priifer ring. Next we consider a ring which may not
be Dedekind domain but. is a Priifer ring.
Let R be an integral domain which has the property that given a,b ? R:
either a divides & or & divides a. Such a ring R is called a valuation ring.
Let / be an ideal of R generated by ai, a2; ... , an. n < oo. We can suppose
that T ^ 0 and. then, also that none of the a^'s is zero. Using the defining
property of R it is fairly easy to see that there exists ano;. 1 < i < n. such
that, at | aj for all j. 1 < j < n. Then / is a principal ideal generated by <2j
and I = R. Hence / is projective. This proves that. R is a Priifer ring.
Theorem 8.3.2 If R is left semihereditary, then every finitely generated
left R-submodule of a free left R-module is direct sum of finitely many
finitely generated left ideah of R
Proof. Let A be a finitely generated left i?-submodule of a free left R-
module F. Let \xk \ k ? K} be a basis of F. Every generator of A is a
linear combination of only a finite number of x^s. There being only a finite
number of generators of A. taking the submodule of F generated by all the
basis elements of F which occur in the representations of the generators we
can regard A as a submodule of this submodule F1 of F. The basis elements
of F being linearly independent over R. F\ is a free i?-module. In view of
© 2003 by CRC Press LLC
this we may assume that. F itself has a finite basis, say X\,X2- ¦¦¦ , xn. We
may now prove the result by induction on n.
Tf n = 1. then F = R and. therefore. A is isomorphic to a finitely
generated left ideal of R. Suppose that n > 2 and that every finitely
generated submodule of a free module with basis having m < n — 1 elements
is isomorphic to a direct, sum of finitely many finitely generated left ideals
of R. Take F-\ the free submodule of F with basis xi,X2; ... , ?n-i and
define B = A f~l F-\. Every element a ? A can be uniquely written as
a = b + rxn. b € Fi, r G R. Define a map 6 : A -»¦ R. by 9(b + rxn) = r.
where b ? Fi, r ? R. The map 0 is an i?-homomorphism and its kernel is
precisely B. Let / be the image of 0. Then we get an exact sequence
(8.6) 0->S->ylA/->0
/ being homomorphic image of a finitely generated module is finitely gen-
generated. The ring R being semihereditary. / is projective i?-module and the
sequence (8.6) splits. Thus A = B (B I. The submodule B being direct,
summand of a finitely generated module is finitely generated. Already B is
a submodule of a free i?-module F^ having a basis consisting of n — 1 ele-
elements. Therefore, by induction hypothesis. B is isomorphic to direct sum
of finitely many finitely generated left ideals of R. Hence A is isomorphic
to direct, sum of finitely many finitely generated ideals of R. rj
Theorem 8.3.3 A ring R is left semihereditary if and only if every finitely
generated submodule of a projective R-module is projective.
Proof. Suppose that R. is left semihereditary. Let A be a finitely gen-
generated submodule of a projective i?-module P. Let P be a submodule of
a free i?-module F. Then A is a submodule of F and by Theorem 3.2. A
is isomorphic to direct, sum of finitely many finitely generated left ideals of
R. Each of these ideals of R is projective. Hence A is projective.
Conversely, suppose that, every finitely generated submodule of a pro-
projective module is projective. Now R. being a projective i?-module. every
finitely generated left ideal of R. is projective and R. is left semihereditary.
?
Proposition 8.3.4 If R is an integral domain with Q as its field of quo-
quotients, then every torsion-free R-module can be embedded in a vector space
over Q. If A is a finitely generated torsion-free R-module, then A can be
embedded in a finitely generated free R-module.
Proof. Let A be a torsion-free i?-module. Let E be an injective R-
module and <f> : A —> E be a monomorphism. Let tE be the torsion
submodule of E and n : E —> E/tE be the natural projection. Since A
is torsion-free tt<j> : A —> E/tE is also a monomorphism. Now E being
injective is a divisible i?-module. Therefore E/tE is divisible. Also E/tE
© 2003 by CRC Press LLC
is torsion-free. Then E/tE is a vector space over Q (Proposition 3.3.9).
Thus A is embedded in a vector space over Q.
Next suppose that A is finitely generated as well. Choose a basis of the
vector space E/tE. Identifying A with its image under the monomorphism
ntp. every generator of A can be expressed as a linear combination over Q of
a finite number of basis elements of E/tE. Consider all the basis elements
of E/tE which occur in the represeatations of the generators of A. Let V
be the subspace of E/tE spanned by these finite number of basis elements,
say ui,U2; ¦¦¦ ,um. Let A be generated by Oi,O2; ••• ,an. Suppose that for
1 < i < n, cii = ^2j{rij / Sij)uj, where r^, Sij ? R and none of the Sij is zero.
Take s = Yli ,• sij and for 1 < i < n, 1 < j < m, let s'- = s/sij. Then Oj =
^2jrijs'ij(s~1Uj). Thus at belongs to the i?-submodule B of V generated
by the elements s~1Ui,s~1U2, ¦¦¦ ,s~1um. Since «i,U2; ••• ,um are linearly
independent over Q. s~1Ui,s~1U2: ••• , s~1um are linearly independent over
R. Hence B is a finitely generated free i?-module and A is embedded in a
finitely generated free i?-module. rj
Theorem 8.3.5 An integral domain R is a Priifer ring if and only if every
finitely generated torsion-free R-module A is protective.
Proof. Let R be a Priifer ring. Let A be a finitely generated torsion-free
i?-module. Then A is a submodule of a finitely generated free i?-module
(Proposition 3.4). The ring R being a Priifer ring, it follows from Theorem
3.3 that A is projective.
Conversely, suppose that every finitely generated torsion-free i?-module
is projective. R being an integral domain, every ideal of R is torsion-free.
Then by hypothesis, every finitely generated ideal of R is projective and R
is a Priifer ring, rj
8.3.6 Exercises
1. Prove that every homomorphic image of a finitely generated module
is finitely generated.
2. Give an example to show that every submodule of a finitely generated
module need not be finitely generated.
3. If R. is an integral domain, prove that every projective i?-module is
torsion-free.
© 2003 by CRC Press LLC
Chapter 9
Universal Coefficient
Theorem
The aim of the present chapter is to prove the universal coefficient theorems
for homology and cohomology. A special case of the Kunneth formula is
considered.
9.1 Universal Coefficient Theorem for Ho-
Homology
Let (C,d) be a complex of left i?-modules. For an integer n. let Zn(C) =
Ker dn, Bn(C) = Im dn+l and Hn(C) = Zn(C)/Bn(C).
Theorem 9.1.1 If A is a right R-module and (C,d) is a complex of left
R-modules such that
(9.1) Tor?(A,Bn(C)) = 0 = Tor*(A,Zn(C))
for all n, then we hane an exact sequence
(9.2) 0 -> A ® Hn(C) 4 Hn(A ®fl C) A Tor?(A, Hn^ (C)) ->¦ 0.
If R is left hereditary and C is a protective complex, then the sequence (9.2)
is exact and splits.
Proof. For any n. let B'n(C) = _Bn_i(C). Then we have short exact
sequences
(9.3) 0 4Zn(CLC^^(CL 0
where dn is the homomorphism induced by dn. Since Tor^(A,B'n(C)) = 0
for every n. the above sequences on tensoring with A over R yield exact
203
© 2003 by CRC Press LLC
sequences
0 -> A ®R Zn(C) ^ A ®R Cn l^n A <g>fl s;(C) -> 0.
Taking Zn+l(C) -> Zn(C) and B'n+l(C) -> -B^(C) to be zero maps we get
an exact sequence of complexes
>C->?'(C) ->0
which on tensoring with A over B. gives an exact sequence of complexes
(9.4) 0^ A®R Z(C) -> A ®R C -> yl ®R B'(C) -> 0.
Since the chain maps on Z(C) and -B'(C) are the zero maps, long exact
sequence for homology for the exact sequence (9.4) gives an exact sequence
A®RBn(C) 4 A®RZn(C)^Hn(A®RC)^A®RBn-1(C)
4 AtonZn-^C)
where d is the connecting homomorphism. A close look at the definition of
the connecting homomorphism corresponding to the exact sequence (9.4)
of complexes shows that, d : A ®r Bn(C) —> A ®r Zn(C) is the same as
the homomorphism 1a® i where i : Bn(C) —> Zn(C) is the inclusion map.
Consider the commutative diagram
A <g> Bn(C) A <g> Zn(C) A <g> Hn(C)
A <g> Bn(C) A <g> Zn(C) Hn(A <g> C)
0 -Torf (A #„_
© 2003 by CRC Press LLC
with exact rows. The top and bottom rows are parts of the long exact
sequence for Torn(A,—) corresponding to the short exact sequence 0 —>
Bn(C) -4 Zn(C) -»¦ Hn(C) ->¦ 0 where i is the inclusion map. The bottom
row in the above diagram has a 0 on the left because Tor^{A, Zn-X (C)) = 0.
The commutative diagram induces homomorphisms
a : A®RHn{C) -> Hn(A®RC) and A : Hn{A®RC) -> Tor^A,Hn^C))
making the completed diagram commutative. An easy diagram chasing
then shows that, the sequence
0 -> A ®R Hn(C) 4 Hn(A ®R C) A Tor?(A, ffn_i(C)) -> 0
is exact. Observe that, the homomorphism a is defined as follows :
If Yl aj ® (cj + Bn(C)) G A(g>R Hn(C), where Cj G Zn(C) then
aC^2ai ® (ci +Bn(C))) = C^aj <g)Cj) +Im{lA® dn+i).
Since all the homomorphisms in the commutative diagram are natural, the
induced homomorphisms a. f3 are also natural. Hence (9.2) is a natural
exact sequence.
Now suppose that R is left hereditary and C is a complex of projective
modules. Then every submodule of a projective module is projective and.
therefore. (9.1) follow and we have the natural exact sequence (9.2).
Since every B'n(C) is projective, the sequence (9.3) splits. Let jn : Cn -»¦
Zn(C) be a splitting homomorphism. Then jni = identity of Zn(C).
Composing jn with the natural projection pn : Zn(C) -»¦ Hn(C) we get a
homomorphism : Cn —> Hn(C). These homomorphisms then induce a chain
map {1 <& (Pnin)} '¦ A ®r C ->¦ A ®r H(C). Observe that the boundary
operator in the chain A ®r H(C) is the zero map. This chain map then
induces homomorphisms 0 : Hn(A ® C) —> A ® Hn(C) given by:
where X] a« ® c« ^ Ker(l ® dn).
For any a G A. c G Kerdn = Zn(C).
as c G Zn(C). This implies that. 8a = identity map of A (Sir Hn(C).Hence
the sequence (9.2) splits, rj
Proposition 9.1.2 The spliting of the exact sequence (9.2) is natural in
A.
© 2003 by CRC Press LLC
Proof. Of course it is being assumed for this result that R is left heredi-
hereditary and C is a complex of projective modules. We continue to have the no-
notations of the proof of the last theorem. Let A' be another right i?-module
and / : A —> A' be an i?-homomorphism. Then we get a commutative
diagram
A<
A' ®R C
® (Pnln)}
A®RH(C)
A' ®R H(C)
of complexes which induces a commutative diagram
Hn(A®RC)
r
Hn(A'®RC)
A<g>RHn(C)
r
A'®RHn(C)
for every n. To see the commutativity of this diagram, consider an element
]T<2i ® Ci + Im 1 ® dn+i in Hn(A ®R C). Then
) dn+1)) = f*(Z at ® Gn(ci) + Bn))
E(O() )
and
^/*(EK ® Q + Jm(l ® dn+i))
= E /(ai) ® Gn(c<) + Sn) = /*#(E af ® Ci + Jm(l <8) dn+1)).
From this it follows that ^/* = f*9. This proves that the splitting homo-
morphism 0 is natural in A. q
9.2 Universal Coefficient Theorem for Co-
homology
Now, let (A, d) be complex of left i?-modules. For an integer n, let Zn(A) =
Ker dn, Bn(A) = Im dn+l and Hn(A) = Zn(A)/Bn(A).
Theorem 9.2.1 // (A,d) is a complex of left R-modules and C is a left
R-module such that
(9.5) ExtlR(B(A),C) = 0 = ExtlR(Z(A),C)
then we have an exact sequence
0 -> ExtR{Hn_x{A),C) A Hn(Horn(A,C)) 4 Hom(Hn(A),C) -> 0.
(9.6)
© 2003 by CRC Press LLC
If R is left hereditary and the complex A is projective, then the sequence
(9.6) is exact and splits.
Proof. For any n, let B'n(A) = ??ra_i(A). Then we have short exact
sequences
(9.7) 0 -> Zn(A) -4 An H B'n(A) -> 0
where i is the inclusion map and dnis the homomorphism induced by dn.
Since Ext1R(Bn(A),C) = 0 for every n. we get exact sequences
0 -> HornR(B'n(A),C) % HornR(An,C) 4 HomR(Zn(A),C) -> 0.
Taking Zn+i(A) —> Zn(A) and B'n+1(A) —>¦ B^(A) to be the zero maps we
get an exact sequence of complexes
0 -> Z(A) -> A -> S'(A) -> 0
which on applying iJom/j( —, C) gives an exact sequence
(9.8) 0 -> HornR(B'(A),C) ^ HornR(A, C) A HornR(Z(A),C) -> 0
of complexes. Since the chain maps on ^(A) and B'(A) are the zero maps,
long exact sequence for homology for the exact sequence (9.8) gives an exact
sequence
) 4 HorriRiBn^iA)^) -> Hn(HomR(A,C))
(9.9) ->¦ ffomfl(Zre(A),C) 4 HomR(Bn(A),C).
Here 9 is the connecting homomorphism. Consider part of the commutative
diagram which leads to the above exact sequence:
0 -
Hom(Zn(A),C) —0
1 1 1
Let / G Hom(Zn(A),C) and g G Hom(An,C) be such that. / = i*(g) = gi
so that g | Zn(A) = f. Now d*n+1(g) = gdn+l = gidn+l = d*n+1(gi).
Therefore, by the definition of the connecting homomorphism d(f) = gi =
© 2003 by CRC Press LLC
(A)= J \b (A)= l U) where i is the inclusion map : Bn(A) —>¦
g d = i*. the homomorphism induced by the inclusion map i :
.( X\ Thp lnntr pyart spmipiipp for P, Tf.nl— d} rnrrpsnnndinp'
'_ = 9jBn(A)= f lsn(A)= **(/) whl
(P) 9 \b (A) \b (A) (/) ()
Zn{A). Thus d = i*. the homomorphism induced by the inclusion map i :
Bn(A) -»¦ Zn{A). The long exact sequence for ExtR(-,C) corresponding
to the short exact sequence
0 -> Bn(A) -4 Zn(A) 4 #n(A) -> 0
where n is the natural projection yields exact sequences
0 -> HomR(Hn(A),C) ^ HomR(Zn(A),C) U HomR(Bn(A),C)
Since the connecting homomorphism d appearing in the exact sequence
(9.9) equals i* in the above sequences, we get a commutative diagram
ExtR{Hn_y{A),C)
Hn(HomR(A,C))
0 HomR(Hn(A),C)
HomR(Zn(A),C)
HomR(Bn(A),C)
HornR(Zn(A),C)
HornR(Bn(A),C)
in which the rows are exact. This diagram then induces homomorphisms
P : ExtniHn-^A)^) -> Hn(HomR(A,C)) and a : Hn(HomR(A,C))
-»¦ HomR(Hn(A),C) making the completed diagram commutative. A sim-
simple diagram chasing then shows that the sequence
A
Hn(HomR(A,C)) 4 HomR(Hn(A),C) -> 0
is exact. Let us make explicit the way the homomorphism a is defined. Let
/ ? HornR(An,C) such that /dn+1 = 0. Let f = f
y The condition
fdn+1 = 0 implies that / |Bn(A)= / lsn(A)= °- Denne 9 '¦ Hn(A) -> C
© 2003 by CRC Press LLC
by g(a + Bn(A)) = f(a), a G Zn(A). The map g is a well defined R-
homomorphism. Let h G HomR(An_i,C). Then hdn G HomR(An,C) and
(hdn)dn+i = 0. For any a ? Zn(A). hdn(a) = hdn(a) = 0. Therefore g
does not depend on the choice of / ? HomR(An,C) with /dn+1 = 0. We
thus find that for / ? HomR(An,C) with fdn+i = 0 i.e. for / ? Ker d*n+l.
a(f + Imd*n) = g,
where g(a + Bn(A)) = f(a), a G Zn(A).
Now suppose that R is left hereditary and the complex A is projec-
tive. Then every submodule of a protective i?-module being protective.
Bn(A) and Zn(A) are projective for every n and ExtlR(B(A),C) = 0 =
Ext1R(Z(A),C). Therefore we have the exact sequence (9.6).
Letg G HomR{Hn{A),C). Then gir G HomR(Zn(A),C). Since B'n(A)
is projective. the sequence (9.7) splits. Let A : An —> Zn(A) be a split-
ing homomorphism i.e. Xi = identity map on Zn(A) which is the same
as saying that X(a) = a for every a G Zn(A). Take / = girX. Then
girX G HomR(An,C) and girXdn+l = 0. Define
0 : HomR(Hn(A),C) -> Hn(HomR(A,C)) by
g?HomR(Hn(A),C).
The map 9 is a homomorphism and a6 = identity on HomR(Hn(A),C).
This proves that the sequence (9.6) splits.
Let C be another left i?-module and 11 : C —> C be an i?-homomorp-
hism. The homomorphism [i induces homomorphisms /x* : HomR(A, C) —>¦
HomR(A,C) and ^ : HomR(Hn(A),C) -> HomR{Hn(A),C'). It is
fairly easy to check that the diagram
Hn{HomR{A,C')) — HomR{Hn{A),C)
Hn(HornR(A,C')) HornR(Hn(A),C)
is commutative. This proves that the splitting of the exact sequence (9.6)
is natural inC. g
We record without proof two consequences of the Kimneth relations.
The interested reader may refer to Cartan and Eilenberg for details.
Theorem 9.2.2 // A is right R-module, B is an (R, S)-bimodule, C is a
left S-module and the rings R, S are right or left semihereditary, then there
is a natural isomorphism Torf{A,Torf{B,C)) = Torf(Tor^(A,B),C).
© 2003 by CRC Press LLC
Theorem 9.2.3 // A, B, C are as in the above theorem, and the rings R,
S are right hereditary, then there is a natural isomorphism
ExtR(A,Extls{B,C)) =s Extls{Tor*{A,B),C).
9.3 The Knnneth Formula - a Special Case
Let X. Y be chain complexes of right and left i?-modules respectively. We
write d for the boundary homomorphism of both X and Y. For any n, let.
(9.10) (X®flY)n = e Y, Xp®RYi
V+Q=n
and define d : (X ®fl Y)ra+i ->¦ (X ®fl Y)n by defining it on the generators
x <8> y of Xp ® Yq by
(9.11) d(x<E>y)=d(x)®y + (-l)px<E>d(y)., x?Xp,y?Yq.
If x G Xp and y G Yq, then x is said to be of degree p and y of degre q.
Observe that if x G Xp. then d(x) ? Xp-i so that d(x) is of degree p — 1.
Therefore
d(d{x®y)) = d{d{x)®y) + {-l)px®d{y))=d{dx)®y
+ (-l)p-1d(x) ® d(y) + (-l)pd(x) ® 8(y)
+ (-l)p+px® d(d(y)) =0.
Thus X <8)r Y with (X ®r Y)n and d as defined becomes a chain complex.
If x is a cycle in X and y is a cycle in Y. then d(x) = 0 = d(y) and (9.11)
shows that, x ®y is a cycle in X(S>fl Y while if x is a boundary say x = d(z)
and y is a cycle, then x®y = d(z) ® y = d(z®«/) is a boundary. Similarly if
x is a cycle and y is a boundary, then x ® y is again a boundary. Therefore
there is induced a well defined homomorphism
p : #m(X) ®fl #n(Y) ->• ffm+re(X ®fl Y),
p((x + Imdm+i) ®(y + Imdn+i)) =x(g>y + Imdm+n+u x G ifer 9m. y G
Kerdn. There is then induced a homomorphism p : ©?}.ffm(X) ®_r
ff,(Y) -^ Hn(X®RY) where n = m + q. Let Zm(X) = ifer 9m; Sm(X) =
Jm dm+1 and ffm(X) = Zm(X)/Bm(X).
If M is a right i?-module. we can think of M as a complex with differ-
differential zero and M concentrated in degree 0 i.e. Xq = M and Xq = 0 for
q ^ 0. We can then form the complex M ®r Y.
Lemma 9.3.1 If M is flat right R-module, then p : M ®r Hn(Y) —>¦
Hn(M (Sir Y) is an isomorphism.
Proof. Write Zra(Y) = Zn, Bn(Y) = Bn and Hn(Y) = Hn. We then
have a commutative diagram
© 2003 by CRC Press LLC
Yn+1
a
Bn
Hn
0
0
with exact rows and columns. Here d is the homomorphism induced by d
and the other maps are the obvious ones. Tensoring with M over B. gives
a commutative diagram again with exact rows and columns:
M ®fl Y
n+1
M®RBn
0
M ®fl Yn
M®RZn
0
Exactness of the column on the right shows that Ker 1 ® d = M ®r Zn.
Also 1 (g: d : M ®/j l^j+i —> M ®r Bn being an epimorphism. under the
above isomorphism Im A ® d : M ®r Yn+i —> M ®R Yn) is isomorphisic to
the image of M ®R Bn ->¦ M ®r Zn. Hence H(M ®fl Yn+1 - M ®RYn-
M (g)/j Yn-{) = M ®/j Hn. Also observe that this isomorphism is induced
by the map a ® (y + Bn) -> a ® y + Im 1 ® d. a G M, y G Zn. Thus the
isomorphism : M ®r Hn ->¦ Hn(M ®R Y) is p. the homology product, q
Observe that the hypothesis (9.1) of Theorem 1.1 is satisfied if A = M is
a fiat i?-module. Also then Tor^(M, Hn-1(Y)) = 0 and the lemma follows
trivially from Theorem 1.1.
Theorem 9.3.2 (The Kwnneth Tensor Formula) IfY is a complex of
left R-modules and X is a complex of right R-modules such that Zn(X.) and
Hn(X.) are projective modules, for all n, then the homology product
p : 8 Y,m+q=n #m(X) <3r Hq(Y) -> Hn(X ®fl Y) is an isomorphism.
© 2003 by CRC Press LLC
Proof. Write Zn for Zn(X), Bn for Bn(X), Hn for Hn(X) and Dn
for Dre(X) = Xn/Zn ^ 5re_i(X). The i?-module Hn being projective.
the sequence 0 —> Bn —> Zn —> Hn —>¦ 0 splits and then Bn being direct
summand of the projective module Zn is projective. Therefore, for every
n, the exact sequence 0 —> Zn —> Xn —> Dn —> 0 splits. Taking the
boundary operators Zn+i —> Zn and Dn+i —> Dn to be zero for every n,
we can regard Z = {Zn} and D = {Dn} to be complexes. Thus we have
a split exact sequence 0->Z->-X->-D->-0of complexes. Tensoring
this sequence with Y over R. we again get (a split) exact sequence E :
0->Z(g>flY->X(g)ijY->D(g>flY->0. A part of the usual long exact
homology sequence for E gives an exact sequence
Hn+1(D®Y) A-^>+1 Hn(Z®Y) -> Hn(X®Y) -> Hn(D®Y) ^ H^Z^Y)
where Am denotes the connecting homomorphism. Since D is a complex
with 0 boundary operator, the boundary operator for D ® Y is just ±1 ® d
i.e. Dm (8 Yq -> Dm (8 Yq-X is the map (-l)ml (g: dq. The above exact
homology sequence yields for every n an exact homology sequence
(9.12) 0 -> Coker An+1 -> iJre(X ® Y) -> ifer An -> 0.
Let 9' denote the homomorphism : Dm+i —>¦ Zm induced by 9 i.e. d'm+1(x +
Zm+l) = 5m+i(x). X G Xm+i.
For every m. we also have an exact sequence
0 -> Dm+1 -> Zm -> iJm -> 0
which splits. Tensoring this sequence with Hq(Y) and taking direct sum
for rn + q = n, we get an exact sequence
0 -> Jl^m+i ®#,(Y) S^ ^Zm®ff?(Y) ->¦ ^Fm(X) ® ff,(Y) ->¦ 0.
Consider the diagram
f)-^X?Zm®Hq(Y)
P
P
Hn+1(D®Y) -^ Hn{Z®Y)
(9.13)
where the vertical maps p are the homology product maps and both are
isomorphisms (Lemma 3.1).
© 2003 by CRC Press LLC
Consider an element Y(xi + Zm+i) ® (vi + Irn dq+\). where X{ G
Xm+\; Vi ? Ker (dq : Yq —> l^-i). The homomorphism p maps this
element onto the homology class of Y^(xi + Zm+i) ® Vi in Hn+i(D ® Y)
which in turn is mapped under Ara+i onto the element of Hn(Z ® Y) de-
determined by Y^ 9(xi) ® Vi.
On the other hand (d'® 1)(Y,(xi + zm+\)® (vi+Im dq+i)) = Y,d{xt) (&
(vi + Irn dq+i) which under p gets mapped onto the homology class in
Hn(Z (g Y) determined by Yd(xi) <8 vi- This proves that the diagram
(9.13) is commutative. But then Ara+i is a monomorphism for every n
and Coker Ara+1 ^ Cokerd' ® 1 ^ Em+9=re#m(x) ® ^?(Y)- The exact
sequence (9.12) shows that Coker An+1 ^ Hn(X®Y). Hence iJre(X(g)Y) ^
X^m+?=rl Hm(X.)<S>Hq(Y), the isomorphism being precisely the one induced
by the homology product map p. rj
9.3.3 Exercises
1. If B. is left hereditary. A is a right R-module and C is a complex of
left i?-modules. prove that
Tor?(A, B(C)) = 0 = Tor?(A, Z(C))
if and only if Tor?{A, C) = 0.
2. If R is left hereditary. C is a left i?-module and A is a complex of
left i?-modules. prove that
ExtlR(B(A),C) = 0 = ExtlR(Z(A),C)
if and only if Ext^A, C) = 0.
3. Tf A is any Abelian group and C is a complex of Abelian groups,
prove that
Tor? (A, B(C)) = 0 = Tor? {A, Z(C))
if and only if Tor?(A, C) = 0
and
Extlz{B{C),A) = 0 =
if and only if ?x^(C, A) = 0.
4. Let i? be a left hereditary ring and A and C be complexes of respec-
respectively right and left i?-modules with A projective. Prove that there are
natural exact sequences
O^e Y, Hm(A)®RHq(C)^Hn(A,C)
m+q=n
^e Y, Tor?(Hm(A),Hq(C))^0
m+q^n— 1
that splits.
© 2003 by CRC Press LLC
5. Let R be a left hereditary ring, and let. A. C be complexes of left
i?-modules with A projective. Then there is a natural exact sequence
0 -> Uq_m=n+1Ext1R(Hm(A),Hq(C))^Hn(Horn(A,C))
-> Uq^m=nHom(Hm(A),Hq(C)) -> 0
that splits.
© 2003 by CRC Press LLC
Chapter 10
Dimensions of Modules
and Rings
Aim of the present chapter is to introduce projective. injective dimensions
of modules and finally global dimension of rings. It is proved that for a
ring which is both left Noetherian and right Noetherian. the right and left
global dimensions are equal. It is also proved that the left global dimension
of a left artin ring R equals the left projective dimension of R/N, where AT
is the radical of R.
10.1 Projectively and Injectively Equivalent
Modules
Definition 10.1.1 Two i?-modules A and B are said to be projectively
equivalent if there exist projective modules P, Q such that A(QP = B®Q.
Also A, B are said to be injectively equivalent if there exist injective
modules E, E' such that A 8 E = B 8 E'.
Observe that both the relations 'projectively equivalent' and 'injectively
equivalent' defined in RM are equivalence relations (cf. Exercise 1.13A)).
Proposition 10.1.2 Let A, B be projectively equivalent left R-modules, C
be any left R-module and D any right R-module. Then
(a) ExtR(A, C) ^ ExtR(B, C) for all n > 1;
(b) Tor%(D,A) ^ Tor%(D,B) for all n > 1.
On the other hand, if A, B are injectively equivalent, then
(c) ExtR(C, A) ^ ExtR(C, B) for all n > 1.
Proof. We prove only (c) leaving (a) and (b) as exercises. The mod-
modules A. B being injectively equivalent, there exist injective modules X.
215
© 2003 by CRC Press LLC
Y such that. A®X<^B®Y. Now, for all n > 1. ExtR(C, A ffi X) ^
ExtnR{C,A) ®ExtnR{C,X) ^ ExtnR{C,A)., as ExtnR{C,X) = 0, X being
injective. Similarly, for n > 1. ExtR(C,B® Y) ^ ExtnR{C,B). Therefore
ExtR(C, A) ^ ExtR(C, 4
for all n > 1. q
^ ExtR(C,
^ ExtR(C, B)
Theorem 10.1.3 (Schanuel's lemma) Given exact sequences
0 -> if [ 4 A 4 A -> 0 and 0 -> if2 ^ ^2 A B -> 0 w^ere Pj. P2 are
projective modules and A, B are isomorphic, then K-\ ffi Pi — K2 ffi P-\ ¦
Proof. Let a : A -»¦ /? be the given isomorphism. The module p
being projective and ir being an epimorphism, there exists a homomorphism
/3 : Pi —> Pi such that. tt/J = ap. Then there exists a homomorphism
7 : ifi —> K<i such that the diagram
P
A
a
A0.1)
B
0
ffi Pi, ^ : -^2 ffi P —>¦ P2 by
is commutative. Define a map 9 : K^ —
6{a) = (-y(a),i(a)). a ? K^ and
<j>(b,x) =j(b)-/3(x), b?K2, xGPi.
Both 9. (j> are i?-homomorphisms, 9(a) =0 implies that i{a) = 0 and i being
a monomorphism a = 0. Thus (9 is a monomorphism. For a ? Ki, cf?(a) =
(/>G(a), i(a)) = jj(a) — j3i(a) = 0, the left hand square in the diagram A0.1)
being commutative. Therefore Im9 C Ker<f>. Let (b, x) ? K2 © P such
that 0 = <]>{b,x) = j(b) - fi(x). Then ap(x) = tt/3(x) = nj(b) = 0 and
a being an isomorphism. p(x) = 0. Therefore x = i(a) for some a ? KY.
Also j(b) = f3(x) = f3i(a) = j^{a) which implies that, b = 7@). Thus
(b,x) = G@),i(a)) = 9(a). This proves that Kercf> C Im9 and. therefore.
Im9 = Kercj). Next, let y ? Pj. The maps a. p being onto, there exists
x ? p such that n(y) = ap(x) = n/3(x). Therefore y = /3(x) + j(b) for
some b ? Ki and. so. y = <j>(b, —x). Hence <j> is onto. We have thus proved
that, the sequence
A0.2)
0 -> K, 4 K2 ffi P 4 P2 -> 0
is exact. The module P2 being projective. the sequence A0.2) splits which
means that. Ki ffi P2 ^ if2 ffi Pj. n
© 2003 by CRC Press LLC
Corollary 10.1.4 The modules KY and Ki as in Theorem, 1.3 are projec-
tively equivalent.
There is a generalization of Theorem 1.3 and. so also, is of Corollary 1.4
Theorem 10.1.5 Given exact sequences
0 -> K^ -> Pn -> Pn_j -> • • • -> Po -> A -> 0 and
0^K2^Qn^ Qn_! -> • • • -> Qo -> /? -> 0
in which Pi, Qj are protective and, A, B are isomorphic, then
Kl®Qn® Pn-1 © Qn-2 © • • • = K2 © Pn © Qn_i © Pn_2 © • • • ¦
Proof. Exercise (follows by induction on n).
Corollary 10.1.6 The modules K\ andKi in Theorem, 1.5 are protectively
equivalent.
Let A be an i?-module and P : > Pn H Pn-X -> > Po -> A -> 0
be a projective resolution of A. For n > 0. let Kn = Imdn+\. Then Kn
is called an nth syzygy of A. Since A can have more than one projective
resolutions. A can have more than one sets of syzygies. As an immediate
consequence of Corollary 1.6. we have the following
Proposition 10.1.7 Let A be an R-module and, \Kn}, {K'n} be two sets
of syzygies of A. Then Kn and K'n are protectively equivalent.
We next prove results corresponding to Theorems 1.3 and 1.5 for injec-
tive modules.
Theorem 10.1.8 Given exact sequences 0^-AA-E'^L'^-O and
0 -»¦ B ^> E" A- L" -»¦ 0 where E', E" are injective modules and, A, B are
isomorphic, then E' © L" = E" © L'.
Proof. Let a : A —> B be a given isomorphism. The module E" being
injective and i being monomorphism. there exists a homomorphism /3 :
E' —> E" such that /3i = ja. But then there exists a homomorphism
7 : L' —> L" making the diagram
A0.3) 0
A
a
B
© 2003 by CRC Press LLC
commutative. Define maps 9 : E' ->¦ E" © L' and cf>: _E" © L' ->¦ L" by
6»(x) = (/?(x),p(x)); x G
cj>(y, a) = n(y) - 7(a); y G ?"; a G L'.
Check that 9 and <j> are homomorphisms and the sequence
A0.4) 0-^E'AE"ffli'4 L" -> 0
is exact. The module E' being injective. the sequence A0.4) splits and
E' © L" ^ ?" © L'. n
Corollary 10.1.9 TTse modules L'. L" as in Theorem, 1.8 are injectively
equivalent.
Theorem 10.1.10 Given exact sequences 04i4l°4l'->"' -»¦
Xn 4 L -> 0; 0 -> B 4 Y° ^ Yl -> • • • ^ Yn 4 M -> 0 in wfticft ewery
X*. F^ is injective and A, B are isomorphic, then L © Yn © X™ © • • ¦ =
MffiX"©!"™©---.
Proof. We prove the result by induction on n, the case n = 0 being
the result, of Theorem 1.8. Suppose that, n > 1 and that, the result, of the
theorem holds when the number of injective modules in either sequence is
<n. _
_ Let L° = Kerd1 = ImdP, M° = KerS1 = ImS° and d° : X° -> L°,
5° : Y° —> M° be the homomorphisms induced by d°. 5° respectively. Let
i : L° —> X1, j : M° —> Y1 be inclusion maps. Then we get exact sequences
A0.5) 0->44l°^L°->0; 0->/?4r°^>M°->0
and
A0.6) 0^L°Axl ^X2 -> >X"->i->0
(io.7) o -> m° 4 r1 ^> r2 -> —> rn -> m -> o
Let d1: r° © X1 -^ X2. S1: X° © F1 -^ Y2 be the homomorphisms denned
by
d1 (y,x) = dl(x)., y?Y°: x G X1 and
tfi (x,y) = E1(y); xGX°; j/ G F1.
The exact sequences A0.6) and A0.7) then lead to exact sequences
o -> y° © l° DJ) r° © x1 41 x2 4 > xn 4 z, ->¦ o
© 2003 by CRC Press LLC
0 -> X° © M° {14] X° © F1 ^> Y2 $ > Yn -4 M -> 0.
Theorem 1.8 applied to the exact sequences A0.5) shows that X° © M° =
y° ffi L° and then the induction hypothesis applied to the above two se-
sequences implies that.
L ffi Yn ffi X™ ®--- = M ®Xn® Yn~l ©•••¦?
Corollary 10.1.11 Modules L and M as in Theorem 1.10 are injectively
equivalent.
Let A be an i?-module. Choose an injective resolution
For n > 0. let Ln = kerdn. The module Ln is called the nth cosyzygy of
A. The module A can have more than one injective resolutions and. so. A
can have more than one sets of cosyzygies. However, by Corollary 1.11. we
have
Corollary 10.1.12 Any two nth cosyzygies of an R-module are injectively
equivalent.
10.1.13 Exercises
1. Prove that the relation of two modules being projectively equiva-
equivalent is an equivalence relation and so is the relation of two modules being
injectively equivalent.
2. Let 0->i4/?->c4Dbean exact sequence and M be an
i?-module. Prove that the sequence
Q ^ A® M(a4] B ® M A C A D,
where j3 (b,m) = C(b). b G B. m G M. is again exact.
3. Let 44B4c4fl->0bean exact sequence and M be an
i?-module. Prove that, the sequence
where /?(&) = (/3F), 0). b G B. is again exact.
4. If A. B are projectively equivalent right i?-modules and C is any left
^-module, prove that Tor%(A, C) ^ Tor*(B, C) for all n > 1.
5. Prove Proposition 1.2 (a) and (b).
6. Prove Theorem 1.5.
© 2003 by CRC Press LLC
10.2 Dimensions of Modules and Rings
Definition 10.2.1 A left i?-module A is said to be of projective dimen-
dimension < n if there exist a projective resolution P of A with Pm = 0 for
all m > n. If projective dimension of A is < n and there is no projective
resolution X of A satisfying Xm = 0 for all m > n. then the module A is
said to be of projective dimension n. We write ipdim(A) or even lpd(A)
for projective dimension of A. If there is no n with lpd(A) = n, we say
that projective dimension of A is infinity and write lpd(A) = oo. We define
projective dimension of the zero module to be —1.
If B is a right i?-module. we can define rpd(B) - right projective
dimension of B similarly. Observe that a non-zero left i?-module (or right
i?-module) A has projective dimension 0 if and only if A is projective.
Definition 10.2.2 Let A be a left i?-module. A is said to have (or be of)
injective dimension < n if there exists an injective resolution E : 0 —> A —>
E° -> E1 -> • • • of A with Em = 0 for all m > n. Tf A is of injective
dimension < n and there is no injective resolution Y : 0 —> A —> Y° —>
Yl —> ¦ ¦ ¦ of A with Ym = 0 for all m > n. then A is said to have left
injective dimension n. We write lid(A) for injective dimension of A. If
there is no non-negative integer n with lid(A) = n. we say that, injective
dimension of A is oc and write lid(A) = oo. We define injective dimension
of the zero module to be —1.
We can define right injective dimension rid(B) of a right i?-module
similarly. Also observe that, a non-zero left (or right) i?-module A has
injective dimension 0 if and only if A is an injective module.
It is more common to work with projective dimensions of modules in
detail and leave the study of injective dimensions. Here we adopt the
opposite path.
Theorem 10.2.3 For a left R-module B, the following are equivalent.
(a) lid(B) < n;
(b) ExtkR{A, B) = 0 for all left R-modules A and for all k > n + 1;
(c) Ex??l{A,B) = 0 for all left R-modules A;
(d) Every injective resolution of B has an injective (n-l)th cosyzygy.
Proof. Let lid(B) < n. Then there exists an injective resolution E
of B with Ek = 0 for all k > n + 1. For any left i?-module A and any
k > n + L ExtkR{A,B) ^ Hk(HomR(A,EB)) = H(HomR(A,Ek-1) -
HomR(A,Ek) - HomR(A,Ek+1)) = 0; Ek being 0. Thus (a) implies (b).
That (b) implies (c) is trivially true. Suppose that Ext'R+l (A, B) = 0 for
all left i?-modules A. Let Lk denote kth cosyzygy of B for some injective
resolution E of B. By Theorem 6.2.4 and Corollary 6.1.3 it. follows that.
© 2003 by CRC Press LLC
ExtlR{A,Ln-1) ^ ExtR+l(A,B) = 0. Therefore, every extension of Ln~l
by A splits for every left /^-module A which implies that Ln~l is an injective
module.
Next, suppose that every injective resolution of B has an injective (n -
l)th cosyzygy. Consider an injective resolution E of B. Let Ln~1 be (n —
l)th cosyzygy of E Then the sequence
0 -> B -> E° -> E1 -> > S™ -> L™ -> 0
is exact and. by hypothesis. Ln~1 is injective. Therefore, it. is an injective
resolution of B and lid(B) < n. q
Corollary 10.2.4 In order that ExtlR(A,B) = 0 for all left R-modules A
it is necessary and sufficient that B be an injective module.
Proof. The zero module being always injective and also ExtlR(A,B)
being zero for all A when B = 0 we may assume that. B ^ 0. Now B is
injective if and only if id(B) = 0 which is so if and only if ExtlR(A, B) = 0
for all left i?-modules A. q
Proposition 10.2.5 A left R-module B has injective dimension < n if and
only if ExtR(—,B) is a right exact functor.
Proof. Let lid(B) < n. Long exact sequence for Ext'R(-,B) corre-
corresponding to a short exact sequence 0 -»¦ A' -»¦ A -»¦ A" -»¦ 0 yields an exact
sequence
ExtE{A",B) -> ?;atf?(A, B) -> ExtE{A', B) -> JBx^+I(A, B) = 0
(by Theorem 2.3). Hence ExtR(-,B) is right exact.
Conversely, suppose that ExtR(-,B) is right exact. Let E be an injec-
injective resolution of B and Lk be the fc?/i cosyzygy of E. For k > 0. we then
get exact sequences
0^ Lk~l -^ Ek ^ Lk ^ 0.
For any left i?-module A, we get an exact sequence
A0.8) HomR(A,Ek) -> HomR(A,Lk) ^ ExtlR(A,Lk-1)
and the module ?* being injective _Ex^(A,_E*) = 0. Also
^ ?z4+1(A?) (Theorem 6.2.4 and Corollary 6.1.3). Let A, A' be left
i?-modules with a monomorphism a : A' —»¦ A. The exact sequences A0.8)
being natural, we get a commutative diagram
© 2003 by CRC Press LLC
HomR{A,En-r) HomR(A,Ln-1) - Ext'R(A,B)
HomR{A',En-r) *¦ HomR{A',Ln-r) *¦ Ext'R(A',B) - 0
with exact rows. The module En~l being injective. the homomorphism
a* : HomR(A,En~l) -»¦ HomR(A',En~1) is an epimorphism and a* :
ExtR(A,B) —> ExtR(A',B) is an epimorphism by hypothesis. Therefore
the homomorphism a* : HomR(A,Ln~l) —> HomR(A',Ln~l) is an epi-
epimorphism showing that Ln~1 is an injective module and lid(B) < n. q
Proposition 10.2.6 Let 0 -»¦ B' ->¦ i? ->¦ B" ->¦ 0 fte an eznr:* sequence of
left R-modules with B injective. Then either all three modules are injective
orid(B') =id(B") + l.
Proof. If B' is injective. the given sequence splits and B" being direct,
summand of an injective module is injective. Suppose that. B' is not injec-
injective. Then id(B') > 0. Then B" ^ 0. Let id(B") = n. For any module
A and k > 1. the long exact sequence for ExtR(A, —) corresponding to the
given short, exact sequence implies
A0.9) ExtkR(A, B") =s ExtkR+l (A,B')
which implies that ExtR+2(A,B') = 0. Therefore id(B') < n + 1 Ifid(B') <
n, then ExtR+1(A, B') = 0 and it follows from A0.9) that ExtR(A, B") = 0
for every A showing that. id(B") < n — 1 which is a contradiction. Hence
id(B') = n + 1 = 1 + id(B"). n
Proposition 10.2.7 // 0 -»¦ B' ->¦ B ->¦ B" ->¦ 0 be an exact sequence of
left R-modules and id(B') < n, id{B") < n, then id(B) < n.
Proof. By given hypothesis, ExtR+l(A,B') = ExtR+l(A,B") = 0 for
every left i?-module A. The long exact sequence for ExtR(A,-) corre-
corresponding to the given short exact sequence then implies that ExtR+l (A, B) =
0 for every module A. Therefore id(B) < n. q
We next state without proof results for projective dimensions of modules
corresponding to the results obtained for injective dimensions.
Theorem 10.2.8 For a left R-module A, the following conditions are equiv-
equivalent :
(a) lpd(A) < n;
(b) ExtkR+1 (A, B) = 0 for all k > n and all left R-modules B;
(c) ExtR+1 (A, B) = 0 for all left R-modules B;
(d) every projective resolution of A has projective (n-l)th syzygy.
(e) the functor Ext'R(A, -) is right exact.
© 2003 by CRC Press LLC
Corollary 10.2.9 In order that ExtlR(A,B) = 0 for all left R-modules B
it is necessary and sufficient that A be a projective module.
Proposition 10.2.10 Let 0 -»¦ A' -»¦ A -»¦ A" -»¦ 0 6e an exact sequence of
left R-modules with A projective. Then either all three modules are projec-
projective or lpd(A") = 1+ ipd(A').
Proposition 10.2.11 If 0 -> A' -> A -> A" -> 0 be an exact sequence of
left R-modules and pd(A') < n, pd(A") < n, then pd(A) < n.
Proposition 10.2.12 If A is a left R-module with pd(A) = n < oo, then
there exists a free R-module F with ExtR(A,F) ^ 0.
Proof. Suppose that Ext'R(A, F) = 0 for every free i?-module F. Let B
be a left i?-module. Then B = F/Kwheve F is a free i?-module and K is
a submodule of F. The long exact sequence for Extu(A, -) corresponding
to the sequence 0—> K —> F —> R —> 0 yields an exact sequence
0 = ExtR(A,F) -> ExtR(A, B) -> ExtnR+l{A, K) = 0
which implies that ExtR(A, B) = 0. Since it is true for arbitrary B, pd(A) <
n-\ (Theorem 2.8) which is a contradiction, q
10.2.13 Exercises
1. Let 0 -»¦ A' -»¦ A ->¦ A" ->¦ 0 be an exact sequence of left R-modules.
(a) If id(A') < n, id(A") = n, then id(A) = n:
(b) lipd(A') = n. pd(A") < n. then pd(A) = n.
2. If m > 2 is an integer, prove that, the projective dimension of the Z-
module Z/mZ is always 1. What can you say about the injective dimension
of Z/mZl
3. Tf B is a left R-module with id{B) < n < oo. prove that there exists
an injective module E such that Ext'R(E,B) ^ 0.
4. Recall that a left R-module A is cyclic if there exists an element
a G A such that. A = Ra = {ra \ r G R}. Prove that, a left R-module
E is injective if and only if ExtlR(A, E) = 0 for every cyclic R-module A.
(Compare it. with Lemma 3.3 to follow).
5. Prove that an R-module B has lid(B) < n if and only if ExtR+l (A, B)
= 0 for all cyclic R-modules A.
6. Let 0 -»¦ A' -»¦ A -»¦ A" -»¦ 0 be an exact sequence of left (right)
R-modules. If two of the modules A', A, A" have finite left (right) projec-
tive/injective dimension, then the third module also has finite left (right)
projective/injective dimension.
© 2003 by CRC Press LLC
10.3 Global Dimension of Rings
Definition 10.3.1 The left protective global dimension lpD(R) of a
ring R is defined by
lpD(R) = sup\lpd(A) \Ae RM}
and the left injective global dimension UD(R) of R is defined by
UD{R) = sup{lid(A) \ A G RM}.
Theorem 10.3.2 lpD(R) = UD(R) for any ring R.
Proof. Let lpD(R) = n < oo. Then lpd(A) < n for all A G RM.
Therefore, ExtnR+l(A,B) = 0 for all left i?-modules B. Hence
ExtnR+l(A,B) =0 for all A, B ? RM.
In other words, for every B G RM, ExiR+l{A,B) = 0 for all A G RM.
This implies that. lid(B) < n for all B G rM or that. lid(R) < n.
On the same lines it. follows that, if UD(R) < m. then lpD(R) < m.
Hence UD(R) = lpD(R) = n.
Next, suppose that. lpD(R) = oo. If UD(R) = m < oo. as observed
above. lpD(R) < m < oc which is a contradiction. Therefore UD(R) = oo.
?
Tn view of the result above distinction between left projective global
dimension and left injective global dimension of a ring disappears, and the
common value is called the left global dimension of R. and is denoted by
We can also similarly define right global dimension rD (R) of R. The
two global dimensions of R are not always equal but. we do not consider
this question here.
We need the following simple characterization of injective modules for
getting a characterization of global dimension of rings.
Lemma 10.3.3 A left R-module B is injective if and only if ExtR(R/I, B)
= 0 for every left ideal I of R.
Proof. Tf B is injective. then ExtlR(A,B) = 0 for all A GR M. Tn
particular. ExtlR(R/I,B) = 0 for all left ideals / of R. This is so because
for a left ideal / of R. the quotient group R/I becomes a left i?-module.
Conversely, suppose that. ExtlR(R/I,B) = 0 for all left ideals / of R.
Let / be a left ideal of R and consider the initial part of the long exact
© 2003 by CRC Press LLC
sequence for ExtR(—,B) corresponding to the exact sequence 0 —> I A
R —> R/I —$¦ 0. We get an exact sequence
0 -> HomR(R/I,B) -> HomR(R,B) ^ HomR(I,B) -> ExtR(R/I,B) = 0
Thus i* is an epimorphism showing that every homomorphism from I to B
can be extended to a homomorphism from R. to B. It follows from Baer's
Criterion for injectivity (Theorem 3.3.5) that B is an injective module, q
Theorem 10.3.4 For any ring R, ID(R) = Sup{pd(R/I) \ I a left
ideal of R}.
Proof. Let T be a left ideal of R. Then R/I is a left i?-module. Thus
{R/I | / a left ideal of R} C RM and Sup{pd(R/I) \ I a left ideal of R}
< Sup{pd{A) \AgrM}=W(R).
If Sup{pd(R/T) | / a left ideal of R}= oo, then as above ID(R) = oc and
we are through. Suppose that Sup{pd(R /1) \ T a left ideal of R}= n < oo.
Then ExtR+l (R/I, B) = 0 for all left ideals I of R and all B G RM. Let B
be a left i?-module and Ln~l be (n — l)th cosyzygy of an injective resolution
of B. Then ExtR{R/I,Ln-1) ^ ExtR+l(R/I,B) = 0 for every left ideal
/ of i?. It follows from Lemma 3.3 that. Ln~1 is an injective module and.
then, id(B) < n (by Theorem 2.3). Thus id(B) < n for all B G RM and,
therefore, W(R) < n. Since Sup{pd(R/I) \ T is a left ideal of R) = n.
there exists a left ideal T of R. with pd(R/T) = n. Thus, there exists a left
R-module A (which is R/I) such that. pd(A) = n. Hence ID(R) ft n and
W{R)=n.u
Corollary 10.3.5 For any ring R, ID(R) = Sup{lpd(A) \ A cyclic left
R-module}.
Proof. If A is a cyclic left i?-module. let a G A such that A = Ra. The
map r —> ra. r G R. is an epimorphism of left i?-modules and if T denotes
the kernel of this homomorphism. then T is a left ideal of R. and R/I = A.
On the other hand for any left ideal T of R. R/I is a cyclic left i?-module
generated by 1 + /. 1 the identity element, of the ring R. This proves that.
a left i?-module A is cyclic if and only if A = R/I for some left ideal / of
R and the corollary follows from the above theorem, q
Corollary 10.3.6 If ID(R) > 1, then ID(R) = 1 + sup{lpd(I) \ I a left
ideal of R}.
Proof. Since W(R) > 1. there exists a left ideal T of R. for which
lpd(R/T) ^ 0 and. therefore. R/I is not projective. It follows from Propo-
Proposition 2.10 that lpd(R/I) = 1 + lpd(I). By Theorem 3.4,
W(R) = sup{pd{R/J) | J a left ideal of R]
= sup{pd(R/J) | J a left ideal of R for which R/J is not projective}
© 2003 by CRC Press LLC
= sup{l + pd(J)
= 1 + sup{pd(J)
= 1 + sup{pd(J)
J a left ideal of R for which R/J is not projective}
J a left ideal of R with R/J not projective}
J a left ideal of R}.
as when R/J is projective. then J is also projective and pd(J) = 0. q
Projective/injective dimension of a module is determined through Ext
and Tor does not occur anywhere. However, we can define a certain di-
dimension of modules using Tor.
Definition 10.3.7 A right .R-module A is said to have weak right ho-
homological dimension < n if Tor^+1 (A, B) = 0 for all left .R-modules B.
We express it by writing w.r.dh(A) < n. The module A is said to have
weak right homological dimension n if Tor^+1(A,B) = 0 for all left R-
modules B and there exists a left .R-module B for which Tor^(A,B) ^ 0.
We then write w.r.dh(A) = n. If there is no non-negative integer n for
which Tor^+1(A,B) = 0 for all left .R-modules B. we say that weak right
homological dimension of A is infinity and we write w.r.dh(A) = oo.
Definition 10.3.8 A left .R-module B is said to have weak left homog-
ical dimension < n if Tor^+1 (A, B) = 0 for all right .R-modules A. We
then write w.l.dh(B) < n. Tf w.l.dh(B) < n and w.l.dh(B) ¦? n. we say
that weak left homological dimension of B is n and we write w.l.dh(B) = n.
If there is no non-negative integer n such that. w.l.dh(B) < n. then we
say that, weak left homological dimension of B is infinity and we write
w.l.dh(B) = oo.
Left (right) projective/injective dimensions of modules can be charac-
characterized through projective/injective resolutions. Weak right (left) homolog-
homological dimension can be characterized through certain resolutions called flat
resolutions. Also then results analogous to Theorems 2.3 and 2.8 can be
obtained and for doing so we have to prove that. Tor can be defined using
flat resolutions in place of projective resolutions of modules. We do not
pursue this point any further.
Since flatness is a weaker condition than being projective, the dimension
obtained by using Tor which can be defined through flat resolutions is called
weak homological dimension in comparison with the dimension which uses
Ext and hence projective resolutions.
Lemma 10.3.9 Let B be a left R-module and n, > 0 be an integer such thai
Tor*(A,B) = 0 for all right R-modules A. Then Tor*+1(A,B) = 0 for all
right R-modules A.
Proof. Let A be a right .R-module. Consider an exact sequence 0 —>¦
A' —> P —> A —> 0 where the module P is projective. The long exact
© 2003 by CRC Press LLC
sequence for Tor(—,B) corresponding to this sequence gives an exact se-
sequence
Tor*+1(P,B) -> Tor*+1(A,B) -> Tor«(A',B)
in which the first term vanishes because P is protective and n + 1 > 1. and
the third term vanishes by hypothesis. Therefore Tor^+1(A,B) = 0. As A
was chosen arbitrarily, we have Tor^+1(A,B) = 0 for all right -R-modules
A.u
On the same lines we can also prove
Lemma 10.3.10 Let A be a right R-module and n > 0 an integer such
that Tor%(A,B) = 0 for all left R-modules B. Then Tor%+1(A,B) = 0 for
all left R-modules B.
Tn view of the two simple observations above w.r.dh(A) for a right B-
module A and w.l.dh(B) for a left .R-module B may be defined as
(i) w.r.dh(A) = the smallest, non-negative integer n. if it exists, such that.
TorR(A, C) = 0 for all k > n + 1 and all left modules C;
(ii) w.l.dh(B) = the smallest non-negative integer n. if it exists, such that
TarR{C, B) = 0 for all k > n + 1 and all right .R-modules C.
Observe that given a right .R-module A, Tor§(A,B) = A (giR B = 0
for all B ? rM. if and only if A = 0. We may thus define the weak
right homological dimension of the zero module to be — 1. Similarly, the
weak left homological dimension of the zero module is defined to be — 1.
Alternatively, if we can allow n. in the definition of w.r.dh(A) or w.l.dh(B)
to be > -1. then w.r.dh(A) = -1 if and only if A = 0 and w.l.dh(B) = -1
if and only if B = 0.
Suppose that sup{w.r.dh(A) | A ? M-r} = n < oo. Then Tor^+1 (A, B)
= 0 for all A G M-r and all B G RM. This implies that w.l.dh(B) < n for
every B G rM and. therefore. sup{w.l.dh(B) | B G rM} < n. Similarly
sup{w.l.dh(B) | B G rM} = m < oc implies that. sup{w.r.dh(A) | A G
Mn) < rn. This proves that sup{w.r.dh(A) | A G .Mk} = sup{w.l.dh(B) |
Definition 10.3.11 The common value sup{w.l.dh(B) | B G .R.M} =
sup\w.r.dh(A) \ A G .Mk} is defined to be weak global dimension of
the ring R and is denoted by w.D(R).
10.4 Global Dimension of Noetherian Rings
Our aim here is to prove that rD(R) = W(R) for a ring R which is both left
as well as right Noetherian. For this purpose we need to define Noetherian
modules and Notherian rings. We also need the concept of weak global
© 2003 by CRC Press LLC
dimension of a ring that, we introduced in the last section (but did not
study in detail).
Definition 10.4.1 A left (right) -R-module A is called Noetherian if ev-
every submodule of A is finitely generated.
Proposition 10.4.2 Every submodule and every quotient module of a Noethe-
Noetherian R-module is Noetherian.
Proof. Since every module is a submodule of itself, every Noetherian
module is finitely generated. Let A be a Noetherian .R-module and A' be
a submodule of A. Then every submodule of A' is a submodule of A and
is finitely generated. Therefore A' is Noetherian. Also any submodule of
A/A' is of the form B/A' where B is a submodule of A and. so. is finitely
generated. But then B/A' is also finitely generated. This proves that A/A'
is Noetherian. q
10.4.3 Examples
1. Every finitely generated Abelian group is a Noetherian Z-module.
2. If R is a principal ideal domain, then every finitely generated R-
module is Noetherian.
3. The additive group of rational numbers is not a Noetherian Z-
module. (Prove it. !)
Definition 10.4.4 A ring R is called left Noetherian if every left ideal
of R regarded as a left i?-module is finitely generated and R is called right
Noetherian if every right ideal of R regarded as a right i?-module is finitely
generated.
The above is equivalent to saying that R. regarded as a left (or right)
.R-module is Noetherian. Tn the case of a commutative ring, the difference
between left and right Noetherian disappears. The ring Z of integers, in
fact any principal ideal domain is a Noetherian ring.
As in the case of rings, a group G every subgroup of which is finitely
generated is called a Noetherian group.
Theorem 10.4.5 The integral group ring ZG is a left (or right) Noetherian
ring if and only if the group G is Noetherian.
Proof. Exercise (cf Ribenboim A969) for details.)
Proposition 10.4.6 Jf0->44M4B->0Bfln exact sequence of left
R-modules and, A, B are Noetherian, then M is Noetherian.
© 2003 by CRC Press LLC
Proof. Let JVbea submodule of M and n(N) = B'. Then tt induces an
epimorphism tt' : AT —»¦??'. Also Kern' = a(A) n AT. Then we get an exact
sequence
0 -> a(A) n]V4Af^B'^0:
where i is the inclusion map. Now B' being a submodule of R is finitely
generated and a (A) fl AT being a submodule of a (A) = A is finitely gen-
generated. Let B' be generated by 61; • • • ,bm and a(A) n Af be generated
by a(a\). ¦¦¦ ,a(ak), where <ii ? A. Choose xi, ¦¦¦ ,xm ? N such that.
Tf'(xi) = bi: 1 < i < m. Let x ? N. Then there exist, ri, ¦¦¦ ,rm
in R. such that ir'(x) = Yl'tLi r^i = SZt=i ri7T'(xi) which implies that
x — Yl'iLi rixi ^ Kern' = a(A) n AT. Therefore there exist s1; • ¦ • , s* in i?
such that x - l^i=l nxi = l_j=1 Sja{aj) or x = l^i=l nxi + l^j=l Sja{aj).
This proves that AT is generated by xi, ¦ ¦ ¦ ,xm,a(a,i), ¦ ¦ ¦ ,a(ak)- Hence
M is Noetherian. q
Corollary 10.4.7 If A, R are Noetherian modules, then so is A © B.
Theorem 10.4.8 If R is left Noetherian and M is a finitely generated left
R-module, then M is Noetherian.
Proof. Let n be the minimum number of generators of M. If n = 1, then
M is cyclic and. therefore. M = R/I. where / is a left ideal of R. Since R
is Noetherian. so is R/I. i.e.. M is Noetherian. Let n > 1 and suppose that
every left .R-module generated by < n — 1 elements is Noetherian. Let M
be generated by ai, a2- • • • , an. Let A be the submodule of M generated
by a,\. Then A is Noetherian by the case n = 1 proved above. Also M/A
is generated by a2 + A, ¦ ¦ ¦ , an + A, i.e.. n - 1 elements. Therefore, by
induction hypothesis. M/A is Noetherian. The sequence 0 —> A —> M —>
M/A —> 0 being exact, it follows from Proposition 4.6 that M is Noetherian.
?
Theorem 10.4.9 Let R be a left Noetherian ring and M be a finitely gen-
generated R-module. Then there exists a resolution F of M in which each Fn
is a free left R-module with finite basis.
Proof. Since M is finitely generated, there exists a free left .R-module
Fo with finite basis and an epimorphism e. : Fq —> M. Let Kq = Kere.
The module Fo is Noetherian (Theorem 4.8) and. therefore. Kq is finitely
generated. Then there exists a free left .R-module F-\ with finite basis and
an epimorphism 5i : -F\ —> Kq. Let Kx = Ker8\. Taking d\ = iSi, where
/ : Kq —> Fq is the inclusion map. we get an exact sequence
0 -> K! -4 FY % Fq 4 M -> 0.
Suppose that we have constructed an exact sequence
A0.10) 0^KnAFnH Fn_! -> ••¦ ->F1^Fo4M->0
© 2003 by CRC Press LLC
in which each Fm is a free i?-module with finite basis. Kn being a submod-
ule of a finitely generated i?-module and R being Noetherian. Kn is finitely
generated. Then, as above, there exists a free left .R-module Fn+\ with
finite basis and an epimorphism 8n+i '¦ Fn+i —> Kn. Let Kn+\ = Ker8n+\
and take dn+\ = i8n+i- We then get an exact sequence
0 -> Kn+1 AF^H1 Fn^ ¦ ¦ ¦ , % Fo 4 M -> 0
extending the exact sequence (lO.fO) by one step. Induction being com-
complete, we get a desired resolution of M. q
Let A, C be left i?-modules and M be a Z-module. For / G Hom(A, M),
r G R, fr-.A-^-M denned by (fr)(a) = f(ra) is a Z-homomorphism and
this makes the Abelian group Horn(A,M) into a right .R-module. Con-
Consider the map 0 : Horn(A,M) x C -»¦ Hornz(Hornn(C,A),M) given by
0(f,c)(a) = f(a(c)), where / G Hom(A,M): c G C and a G HomR(C,A).
The map 0 may be easily checked to be biadditive. Also for / G Hom(A, M).
cGC. r G R and a G HomR(C,A).
9(fr,c)(a) = (/r)(a(c)) = f(ra(c)) = f(a(rc))=9(f,rc)(a):
which proves that 0(fr,c) = 0(f,rc). Therefore. 0 induces a homomor-
phism 0 : Homz{A,M) ®ff C -»¦ Homz(HomR(C,A),M) which is given
by 6{f ®c){a) = f{a{c)), f G Homz(A,M), c G C and a &HomR(C,A).
Proposition 10.4.10 27?,ft map 0 is a canonical, homomorphism and it is
an isomorphism if C is a free left R-module on a finite basis.
Proof. Let a : A' -»¦ A. f3 : C -»¦ C" be .R-homomorphisms and 7 : M -»¦
M' be a Z-homomorphism. The maps a and 7 induce a homomorphism
(a,7)* : Homz(A,M) -> Homz{A',M') which is denned by
For any r G R. f G Homz{A,M), o! G A'.
(a,7)*(/r)=7(/r-)a
and
(a,7)*(/r)(a') = G(/r)a)(a') = 7((/r)(a(a')) = >r(f(ra(a')))
which implies that (a,7)*(/r) = (a,j)*(f)r. Thus (a,7)* is an i?-homomor-
phism and. then we get a Z-homomorphism
(a,7)* ® ^ : Homz(A,M) ®RC -> Homz(A',M') ®R C".
© 2003 by CRC Press LLC
On the other hand a. /3 induce a homomorphism (ft, a)* : HomR(C',A')
—> HomR(C,A) which along with 7 induces a homomorphism
((/?,a)*,7)* : Homz(HomR(C,A),M) -> Homz(HomR(C',A'), M').
Consider the diagram
Homz{A, M) ®R C ° < Homz(HomR(C, A),M)
A0.11) Homz(A',M')®RC ° • Homz{HomR{C',A'),M')
Let / G Homz(A, M). cGC. For any g G HomR(C, A').,
c)(agp) =
lf(ag/3(c)) = (jfagP)(c) and
Therefore, (((/?,«)*,7)*6»)(/ (g) c) = 0((a,j)* (g) /?)(/ ® c) and the diagram
A0.11) is commutative. This proves that. 9 is a canonical homomorphism.
Now suppose that. C is a free left .R-module on a finite basis. Then
C = ffi ^4™ 1 Ci: where d = R. for every i, 1 < i < m. Therefore
HomziA, M)®RC^® Yh=\ Homz(A, M) ®R d and
Homz(HomR(C,A),M) =s 9^=1 Homz(HomR(Ci,A),M).
For any z. 1 < i < m.
Hornz(A, M) (giR Ct = Hornz(A, M)(giRR = Hornz(A, M) under the map
/ ® r -> fr, r G R,f G Homz(A, M) and
Homz(HomR(Ci,A),M) ^ Homz(HomR(R,A),M) ^ Homz(A,M)
the last isomorphism being induced by the isomorphism HomR(R, A) = A
which is defined by g —> (/A); (/ G HomR(R,A). Then the isomor-
isomorphism : Hamz(A, M) -»¦ Hamz(HomR(R,A),M) is given by 5 -*¦<?. 5 G
iJomz(^4, M). where ff (a) = ga(l), a G HornR(R,A).
The diagram
Homz{A,M)®RR ®—+ Homz(HomR(R,A),M)
Homz(A,M)
is commutative showing that 9 is an isomorphism. Thus 9 : Homz(A, M)
®R Ci —>¦ Homz(HomR(Ci,A),M) is an isomorphism for every i and.
therefore.
6» : Hamz(A, M)®RC -> Hamz(HamR(C, A),M)
is an isomorphism. [—j
© 2003 by CRC Press LLC
Lemma 10.4.11 // A-% B —»¦ C is a complex of left R-modules and M is
an injective left R-module, then HomR(Ker/3/Ima,M) = Kera*/Im/3*,
where a* : HomR(B,M) -> HomR{A,M), /3* : HomR(C,M) ->
HomR(B,M) are the homomorphisms induced by a. /3 respectively.
Proof. Let / G Kera* so that fa = 0 and / \ima= 0. Therefore /
induces a homomorphism / : Ker/3 /Ima —> M given by f(b + Ima) =
f(b), be Ker/3. For any g G HomR(C,M), /3*(g) = ~g~J3 and for any
b G Ker/3. gC(b + Ima) = 0. We can thus define a map
4> : Kera*/ImP* ->¦ HomR(KerC/Ima, M),
</>(/ + Imp*) = 7, f G Kera*
which is a homomorphism of ^-modules.
Let g G HomR(KerP/Ima,M). The module M being injective. there
exists homomorphism g-. B/Ima —> M such that g =9 i. where i :
Ker/3/Ima —> B/Ima is the inclusion map. Let tt : B —> B/Ima be
the natural projection. Then 9 ir G Hornn(B, M) and a*(9 ir) =9 ira = 0.
i.e.. 9 n G Kera*. Let 5' : B/Ima -> M he another homomorphism
such that (/'z = g. Then ((/'— ff)i = 0. The module M being injec-
injective . it follows from Proposition 3.2.2 that there exists a homomorphism
h : C ->¦ M such that (g1- 9) = h~J3 where /? : B/Ima -> C is the ho-
homomorphism induced by /?. Now ((/'— (/)tt = /i/?7r = h/3 = /3*(h) showing
that (/'?r + Im/3* =9 tt + Im/3*. Hence 9 tt + Imfi* is indepedent of the
choice of a homomorphism g-. B/Ima —> M satisfying g =9 i. Define
%/> : HomR(Kerf3/Ima,M) -»¦ Kera*/Im.fi* by
^(ff) =5 7T + 7m/3*. g G HamR(Kerj3/Ima, M),
where 9: B/Ima —>¦ M is chosen to satisfy 9 i = g. The map ij) is also a
Z-homomorphism.
Let / G Kera* and </>(/ + Imji*) = /. where / : Ker/3/Ima -> M is
defined by /(& + Ima) = f(b), b G fcer/J. Since fa = a*(f) = 0. / induces
a homomorphism /: B/Ima —>¦ M such that f i = f and f tt = f. This
means that. ?/>(/) =/ n + Im/3* = f + Im/3* showing that ?/)(/> is the identity
map.
Next, let g G HomR(Ker/3/Ima,M) and #: B/Ima 4 Mbea ho-
homomorphism such that. (/ =5 i. Then, by definition. ip(g) =9 tt + Im/3*.
Now a*(9 tt) =9 ira = 0 and it is clear from the definition of (j> that
<j)(g tt + IrnP*) = g showing that. <f>ip is the identity map. Hence <f> is an
isomorphism with ip as its inverse, q
© 2003 by CRC Press LLC
Theorem 10.4.12 If A is a right R-module and B is a left R-module, then
w.r.dh(A) < rpd(A) and w.l.dh(B) < lpd(B).
Proof. We prove that w.l.dh(B) < lpd(B). The result is trivially true
if lpd(B) = oo. So suppose that. lpd(B) = n < oo. Then there exists a
projective resolution P of B with Pm = 0 for all rn > n. For any right
.R-module C and m > n. Tor%(C, B) = H(C ®R Pm+l -C®RPm-C®R
Pm-i) = 0; as P,m = 0. Therefore w.l.dh(B) < n. Q
Lemma 10.4.13 Let R be a left Noetherian ring, C be a finitely generated
left R-module and M an injective Z-module. Then, for any left R-module
A and any n>0, there is an isomorphism Tor^(Honiz(A,M)^C)
^ Homz(ExtR(C,A),M).
Proof. Since C is finitely generated and R is left Noetherian, there exists
a free resolution
F : >FnH Fn_! -> • • ¦ 4 Fo 4 C -> 0
in which every Fn is a free left R-module with a finite basis (Theorem 4.9).
Then, for n > 0 and any A ? rM.
Tor*(Homz(A,M),C) =s Hn(Homz(A,M) ®RFA).
Tn view of Proposition 4.10 we have a commutative diagram
Homz(A, M) ®R Fn+1 <- Homz(A, M) ®R Fn
Homz(HomR(Fn+1,A),M) Homz(HomR(Fn,A),M)
Hornz{A, M) ®R Fn_i
Homz{.HomR(Fn-1,A),M)
A0.12)
with the vertical maps isomorphisms. Therefore Hn(Honiz(A,M) (g)/j
Fc) = homology group of lower sequence in diagram A0.12).
The Z-module M being injective. it follows from Lemma 4.11 that the
homology of the lower sequence in the diagram A0.12) is isomorphic to
Homz(Kerd^+1/ Irnd*n, M) where for any rn > 0, d*m : HomR(Fm-i, A) —>
HomR(Fm,A) is the homomorphism induced by the differential \dm} of
the free resolution F of C. But Kerd*n+1 /Imd*n ^ ExtR(C,A). Also
© 2003 by CRC Press LLC
Hn{Homz{A,M) ®RFC) = Tor^(Homz(A,M),C). Therefore,
TorR(Hamz(A,M),C) ^ Hamz'(ExtR(C,A),M) for every n > 0 and ev-
every A G RM. ?
Theorem 10.4.14 If R is left Noetherian, then wD(R) = W(R) while if
R is right Noetherian then wD(R) = rD(R). In particular, if R is both
right and left Noetherian, then ID(R) = rD(R) = wD(R).
Proof. Suppose that R. is left Noetherian. Since for every left -R-module
A, w.l.dh(A) < l.p.d(A) (Theorem 4.12) we have wD(R) < W{R). There-
Therefore, to prove the first assertion, it is enough to prove that W(R) < wD(R).
liwD(R) = oo. the result follows trivially. Suppose that wD(R) = n < oo.
Then TorR+1(A,B) for every right .R-module A and every left .R-module
B. Let A be a cyclic left .R-module. B be any left .R-module and M be any
injective Z-module. Then
Homz(ExtnR+l(A,B),M) ^Tor*+1(Homz(B,M),A) = 0.
Since every module can be embedded in an injective module, we can find an
injective Z-module M which has ExtR+l(A,B) as a submodule. For this
M, Homz(ExtR+1\A,B),M) = 0 implies that Ext^+1 (A,B) = 0. This
shows that. lpd(A) < n for every cyclic left .R-module A. It then follows
from Corollary 3.5 that. ID(R) < n. Hence ID(R) = wD(R).
The other part follows similarly, q
10.5 Global Dimension of Artin Rings
A left .R-module M is called artinian if every non-empty set of submodules
of M possesses with respect to inclusion ordering a minimal element. This
is equivalent to saying that every descending chain M\ D Mi D • • • of
submodules of M is stationary. A ring R is called a left artin (artinian)
ring if R as a left .R-module is artinian. Our aim here is to prove that left
global dimension of an artin ring R equals the left projective dimension of
R/N, where N is the radical of R.
Observe that the ring Z of integers is Notherian but not an artinian ring.
A finite dimensional algebra over a field is both Notherian and artinian ring.
Let p be prime number and
Qp = {ajp% | a ? Z and i a non — negative integer}.
Then Qv is an artinian but not a Notherian Z-module (cf. Kash for details).
Theorem 10.5.1 Every finitely generated (left) R-module M ^ 0 has a
maximal submodnle.
© 2003 by CRC Press LLC
Proof. Let {mi, • • •, mt} be a system of generators of M. Let
A= {A\ A< M).
Since the submodule 0 is in A. A ^ (j>. Partially order A by inclution. Let
F be a chain (or a totally ordered subset) in A and take
B = UAerA.
The submodule B is an upper bound of F. If B = M. then ni\, • • • ,m,t ? B
and. therefore, there exists an A in F such that, {mi, • • • ,m,t} C A which
gives that M = A. This is a contradiction and B < M or that Be A.
Thus, every chain in A has an upper bound in A. By Zorn's lemma there
exists a maximal element D in A. If there exists a submodule C of M with
D C C < M. then C ? A and D being maximal in A it follows that D = C.
Hence D is a maximal submodule of M. q
Lemma 10.5.2 Let M be a left R-module in which, every subm.odule is a
direct summand. Then M contains a simple submodule.
Proof. If AT is a finitely generated submodule of M. every simple sub-
submodule of N will be a simple submodule of M. Therefore, we can as-
assume that. M itself is finitely generated. Then M has a maximal submod-
submodule A (say). By hypothesis, there exists a submodule B of M such that
M = A e B. This implies that B = M/A and M/A is a simple module
because of A being maximal. Thus M has a simple submodule B. q
Proposition 10.5.3 An R-module M in which, every subm.odule is a direct
summand is semisimple.
Proof. Let A be the collection of all the simple submodules of M.
Lemma 5.2 shows that, there is atleast one simple submodule of M. Let
n = e Y^ Mi-
Then /V is a submodule of M and there exists a submodule A of M such
that M = A ffi N.
If A ^ 0. there exists a simple submodule B of A and. so. a simple sub-
submodule B of M. Then B ? A and. therefore. B C N i.e. B C AnN = 0
which contradicts the above lemma,. Therefore A = 0 and N = M i.e. M
is direct sum of simple submodules. q
Converse of the above lemma is also true.
Proposition 10.5.4 Every subm.od.ule of a semisimple R-module M is a
direct summand of M.
© 2003 by CRC Press LLC
Proof. Let M = © Yiei ^i with each Mi a simple submodule of M.
Let A be a submodule of M. Let (we write X Mi for © XI Mi)
A = {J CI\A
As Z1jg0 Mj = 0. </) e A. Partially order A by inclusion. Let F be a
chain in A. J* = Uj^rJ is an upper bound of F. Let a G A such that
a = X^eJ* -^i- Then there exists a finite subset E of J* such that a =
"^2ieE mi- Since F is a chain, there exists a K G T such that E C K.
Therefore a ? An YieK Mj = 0 and we get a = nn = 0 for all i. This
proves that. A n X^eJ* -^ = 0 an<^ ^* ^ A. By Zorn's lemma, there exists
a maximal element L ? A. Let
We claim that. N = M. For any zn G /, if N n Mio = 0. then zn ^ L and
A n X^iei' Mi = 0; where L' = IU {z0} > L. This contradicts the choice
of L. Thus, for every z'o ? I. N P\ Mi0 ^ 0. Since every Mi0 is simple.
NnMio = Mio or that Mio C TV for every i0 G T. Hence X),e/ Mt C N C M
and M = N = A® Y^teL Mi- D
A ring R is called semisimple if R regarded as a left i?-module is
semisimple. For semisimplicity of a ring R it. is immaterial whether R is
semisimple as a left i?-module or as a right i?-module.
Recall that the radical of a ring R denoted by rad R is the intersection
of all maximal left ideals of R. It is also the intersection of all maximal
right ideals of R. Therefore rod R is a two sided ideal of R. We recall
Nakayama's lemma :
Theorem 10.5.5 A left ideal T of R is contained in rad R if and only if
TM = M implies M = 0 when M is a finitely generated R-module.
Proposition 10.5.6 Let R be a left artin ring. Then we have the following.
(a) The radical of R is nilpotent.
(b) R/rad R is a semisimple ring.
(c) There is only a finite number of nonisomorphic simple R-modules.
Proof. Let us write TV for rad R. Since R is left artin and R D N D
N2 D • • ¦ D Nn D ¦ • - is a decreasing sequence of left ideals, there exists
an n such that. TV" = 7V"+I. Suppose TV" ^ 0. Let A be the class of
all left ideals T of R. with NnT ^ 0. Since 0 ^ Nn = Nn+l = Nn.N,
N G A. Choose a left ideal A in R. which is minimal in A. Choose an
x G A with Nnx ^ 0. Therefore Nn(Rx) ^ 0 and Rx G A. Rx C A. By
the minimality of A. A = Rx which is a finitely generated left ideal. Also
0 ^ NnA = Nn+1A = Nn(NA) and NA C A is in A. By the minimality
© 2003 by CRC Press LLC
of A. NA = A which implies, by Nakayama lemma, that. A = 0. This is a
contradiction. Hence Nn = 0 and AT is nilpotent.
(b) Let / be an ideal of R. with N C I and I/N nilpotent. Then there is
an integer t such that Tl C TV. Since TV" = 0. Is = 0 for s = nt. Let M be
a maximal ideal of R and tt : R —> R/M be natural projection. If / <? M.
then tt(/) ^ 0 in R/M and since R/M is simple. R/M = ir(I). Now
7r(/2) = /„¦(/) = I(R/M) = (IR + M)/M = (I + M)/M = ir(I) = R/M.
Repeating this argument we get 0 = tt(-P) = R/M which is contradiction.
Therefore /CM. Thus / is contained in every maximal ideal of R. There-
Therefore I C N and I/N = 0 in R/N. This proves that. R/N has no non-zero
nolpotent ideal. The ring R/N being homomorphic image of an artin ring
is artin. It follows from (a) above that rad (R/N) = 0. Hence R/N is a
simple ring.
(c) Let A be a simple i?-module. Then A = Ra for some 0/fl?i
and r —>¦ ra. r ? R. gives an isomorphism R/I = A where / is a maximal
left ideal of R. On the other hand, if T is a maximal left ideal of R. then
R/I is a simple i?-module. Thus an i?-module A is simple if and only
if A = R/I for some maximal left ideal T of R. Since N C T for every
maximal ideal / of R. a simple i?-module A becomes an i?/7V-module. A
left ideal I/N of R/N is maximal if and only if / is a maximal left ideal of
R. Since (R/N)/(I/N) ^ R/I, a left iJ-module A is simple if and only if
A is simple as an i?/7V-module. The ring R/N being semisimple and artin.
R/N has only finitely many non-nilpotent minimal left ideals and, therefore,
upto isomorphism only a finite number of simple left i?/7V-modules. Hence
there are. upto isomorphism, only a finite number of simple i?-modules. q
We can now prove the main result, of this section.
Theorem 10.5.7 Let N be the radical of a left artin ring R. Then we have
ID(R) =lpdR(R/N).
Proof. By definition we have ID(R) > lpdn(R/N). This becomes an
equality if lpdR(R/N) = oo. Therefore to prove that. ID(R) < lpdR(R/N),
we may assume that lpdR(R/N) = n < oo. By the previous proposition
there are only a finite number of simple i?-modules and each is a direct
summand of R/N. It follows from Theorem 7.3.5 and 2.8 that lpdR(S) < n
for every simple left i?-module S. We now prove by induction on the length
l(M) of M that. lpdR(M) < n for all finitely generated R-modules M. If
l(M) = 0. M = 0 and we are done. Let l(M) = t > 0. Then there is an
exact sequence
O^S^M^M'^-0
with S a simple left i?-module and l(M') = t - 1 (Lemma 2.1.23). Now
lpdR(S) < n (as seen above) and lpdR(M') <nhy induction hypothesis. It
© 2003 by CRC Press LLC
follows from Proposition 2.11 that. lpdR(M) < n. In particular. lpdR(M) <
n for every cyclic left i?-module M. By Corollary 3.5
ID(R) = sup {lpdR{M) | M cyclic left R - module}
and, therefore, ID(R) < n. Hence ID(R) = lpdR(R/N). D
Corollary 10.5.8 The following are equalent for a left artin ring R.
(a) R is left hereditary.
(b) N is a projective R-module.
(c) pdR(R/N) < 1.
(d) W(R) < 1.
Proof, (a) implies (b) follows from the definition, (b) implies (c) follows
from Proposition 2.10. (c) implies (d) follows from Theorem 5.7. Suppose
that, (d) holds. Let A be a left ideal of R. Then lpdR(R/A) < 1. Propo-
Proposition 2.10 applied to the exact sequence 0 —> A —y R. —y R/A —> 0 shows
that pd(A) = 0. Therefore A is a projective i?-module. Hence R. is left
hereditary, rj
© 2003 by CRC Press LLC
Chapter 11
Cohomology of Groups
In this chapter we study homology and cohomology groups of a group G
with coefficients in a G-module A. For arbitrary G. we describe Hi(G,A),
Hl(G,A), for i = 0, 1 and prove that. Hn(G,A) = Hn(G,A) = 0 for all
n > 1 when G is a free group. We give Bar resolution of a group G and use
it to define cohomology groups of G through cocycles and coboundaries.
The group H2(G, A) is described in terms of group extensions of A by G.
Conditions under which the restriction H2(G,A) —> H2(H,A). when H is
a normal subgroup of G. is zero are studied.
If H is a normal subgroup of G. the cohomology of G is related to the
cohomology of H and that of the quotient group G/H through a spectral
sequence. The low degree terms of this spectral sequence yield a 5-term
exact sequence. We give an elementary proof of this 5-term sequence and
extend this sequence by two terms. Dual of Hopf's formula for H2(G,D).
D a divisible Abelian group is obtained.
11.1 Homology and Cohomology Groups
Let G be a group and ZG be the integral group ring of G. Let A be
an Abelian group written additively. The Z-module A can be converted
into a left (or right) ZG-\noAn\e, by defining the left G-action on A by
xa = a for every x ? G. a ? A and then extending it. to ZG-action by
using linearity. Such a ZG-modu\e A is called a trivial ZG-module. In
particular the group Z of integers can be regarded as a trivial left (or
right) ZG-modu\e. Any left (or right) ZG-modu\e B will, for simplicity,
be called a left (or right) G-module. If A is a right G-module. B, B' are
left G- modules, then we write A <S>g B for A ®ZG B. and HorriG(B,B')
for HomzG(B,B')., Tor°(A,B) for Tor^G(A,B) and ExtG{B,B') for
ExtnZG{B,B>).
239
© 2003 by CRC Press LLC
Definition 11.1.1 Let G be a group and. A be a left G- module. We de-
define the nth ho mo logy group Hn(G, A) and the nth cohomology group
Hn(G, A) of the group G with coefficients in A by
Hn(G,A) = Tor°(Z,A): Hn(G,A) = ExtG(Z,A)
for n > 0 where Z is regarded as a trivial right G-module in the first case
and a trivial left G-module in the second case.
It is clear from the definition that Hn(G, —) and Hn(G, —) are both covari-
ant additive functors from zgM to Ab. In order to compute the groups
Hn(G,A) and Hn(G,A) for a left G-module A. we need to find protective
resolution P of right G-module Z and Q of the left G-module Z. Then, for
n>0,Hn(G,A) = Hn(Pz ®G A) and Hn(G, A) = Hn(HomG(Qz,A)).
Tn view of Theorems 5.3.13. 5.3.15. 6.2.1 and 6.2.4 and the definition of
Hn(G, A) and Hn(G, A) we have
Proposition 11.1.2 Given a short exact sequence 0 —> A' A A —y A" —y 0
of left G-modules , there exist natural exact sequences
H0(G,A') -> H0(G,A)
and
0 _>. H°(G,A')->H°(G,A) ->H°(G,A") -^H\G,A')^ >¦
Hn-l(G,A") -^Hn(G,A') -+ Hn(G,A) -^Hn(G,A") ^
Hn+1(G,A') -+ ¦¦¦
Proposition 11.1.3 For any left G-module A and any n > 2, Hn(G, A) =
ExtG-l{I{G),A) and Hn(G,A) ^ Tor^(I(G),A),' where I(G) denotes
the augmentation ideal of ZG.
Proof. Recall that the augmentation ideal I(G) of ZG is the kernel of
the augmentation hommorphism e : ZG —> Z which is defined by
eB_.nliXi) = yjmi; mi € Z, Xi € G.
The map e is clearly an epimorphism and we get an exact sequence
A1.1) 0-44
with i the inclusion map. Let A be a left G-module. Since ZG is a free
left as well as right G-module. Tor^(ZG,A) = 0 and ExtG(ZG,A) = 0
© 2003 by CRC Press LLC
for all n > 1. The long exact sequences for TorG(—,A) and Exto(—,A)
corresponding to the exact sequence A1-1) then yield
Hn(G,A) = TorG(Z,A)^TorG_l(I(G),A)
and
Hn(G,A))= ExtG(Z,A) =s Ext™-1 (I(G),A) for nil n > 2. n
Observe that for TorG(—, A) A1.1) is to be regarded as exact sequence
of right G-modules while for ExtG(—, A) it is to be regarded as an exact se-
sequence of left G-modules. Observe that we can always choose a G-projective
resolution P of the trivial G-module Z with Po = ZG and e : ZG —> Z the
augmentation homomorphism. Then we get a G-projective resolution P'
of I(G) with P'n = Pn+l for n > 0. Since the definition of Exf%(C, A) and
Tor^(B,C) is independent of the choice of projective resolution of C. we
can get an alternative proof of the above result.
Let G. G' be groups and / : G —> G' be a homomorphism. Extend / by
linearity to a ring homomorphism / : ZG —> ZG'. Let A be a G'-module.
For x ? G. a ? A, define xa = f(x)a. With this A becomes a G-module.
In particular ZG' is a G-module . Indeed ZG' is a (Gr',Gr)-biomodule and
for any left G-module B, ZG' ®g B becomes a left G'-module.
Lemma 11.1.4 // P is a projective left G-module, then ZG' ®g P is a
projective left G' -module.
Proof. Let P be a projective left G-module and consider a diagram
ZG' ®g P
A *- B *- 0
a
of left G'-modules with a an epimorphism. The homomorphism / : ZG —>
ZG' induces a homomorphism / ® 1 : ZG ®G P -> ZG' ®G P of left G-
modules. Also ZG ®g P — P under the G-isomorphism f3(x (g> a) = xa. x ?
ZG. a ? P. Now in the diagram
P
A *¦ B
a
© 2003 by CRC Press LLC
of G-modules a is an epimorphism and P is a projective G-module. There-
Therefore, there exists a G-homomorplism 9 : P —>¦ A such that a9 = g(f®l)j3~l.
The map <f> : ZG' ®G P ^ A given by
<j)(x' (g) a) = x'6(a).. x' ? G', a ? P.,
is a well defined G'-homomorphism and for x' G G'. a ? P.
a<f>(x' (g) a) = a(x'9(a)) = x'(a9)(a) = x'(g(f ®l)/3~l)(a)
= x'g(f (g: 1)A <g> a) = ar'g(l <g> a) = g(a;'(l (g) a))
= g(:r' (g) a).
This proves that acfi = g and ZG' ®g P is a projective G'- module, rj
11.2 Some Examples
(a) Let G be a finite cyclic group of order n generated by x (say). Let
/V = Y1h=q x% ¦ P = x — 1. Consider the complex
A1.2) -> Pm H Pm_! ->¦ • •
where each Pm = ZG. dim. = ^V; <^2m+i = T- Define a contracting homo-
topy s = \sm : Pm —> Pm+i; ^-homomorphisms} by
0 if k = 0
-*> -
0 ifO<k<n-l
Z
The following are then easy to check.
Ts2m + s2m-\N = 1. A^s2m+i + s2mT = 1, Tsq + S-ie = 1 and es-i = 1.
Using these relations we find that A1-2) is an exact sequence and, therefore,
is a G-projective resolution of Z. The complex Homo^Pz, A) on identifying
Homo {ZG, A) = A with A then reads
0 -> An -$¦ AY 4 A2 -> > Am ->•¦-,
where each Ai = A. Therefore, for m > 1.
H2m(G,A) ^ KerT/ImN =s AG/7Vyi;
H2m+l(G, A) ^ KerN/ImT = KerN/I(G)A:
H°(G,A) ^KerT = AG:
© 2003 by CRC Press LLC
where of course AG = {a G A\ga = a for every g G G}. If G acts trivially
on A, then AG = A:NA = nA: KerN = {a G A|na = 0} and I(G)A = 0.
Therefore, in this case, H2m(G,A) ^ A/nA: H2m-l(G,A) = {a G A|na =
0}. H°(G,A) = A. Tn particular, when A = Z. Q or Q/Z regarded as a
trivial G-module. then
(i) H2m(G, Z) ^ ZjnZ, H2'1 (G, Z) = 0, for m>l,
H°(G,Z) ^Z:
(ii) Hm(G, Q) = 0 for all m>l, H°(G, Q) ^ Q:
(in) H2m(G, Q/Z) = 0, H2m-l(G, Q/Z) =s Z/nZ form>l,
Again . since ZG is also a right G-module and ZG ®o A = A, the
complex Pz ®g A reads
> Am -> Am-i -> ¦ • - %¦ Ai -$¦ An -> 0
where each Ai = A. Therefore
H2m(G,A) ^ KerN/ImT = KerN/I(G)A for m > 1;
#2m-i(G,^) =KerT/ImN = AG/NAform> 1;
and
H0(G,A) ^ A/ImT = A/I(G)A.
When A is a trivial G-module. we have H2m(G,A) = {a G A|na =
0}; H2m-i(G, A) ^ A/nA for m > 1 and iJ0(G, A) ^ A.
Definition 11.2.1 If both Ker N/I(G)A andAG/NA are finite groups, we
call
h(A) = \AG/NA\/\KerN/I(G)A\ = \H2(G,A)\/\Hl(G,A)\
the Herbrand quotient of A.
Lemma 11.2.2 If A is finite, then h(A) = 1.
Proof. Since A is finite, the axact sequence
04iG^i^A4 A/I(G)A ->¦ 0
implies that \AG\ = \A/I(G)A\.
The homomorphism TV : A —> A induces an exact sequence
0 ->¦ KerN/I(G)A ->¦ A/I(G)A 4 AG -+ AG/NA -+ 0.
© 2003 by CRC Press LLC
Orders of the groups A/I(G)A and AG being equal.
\KerN/I(G)A\ = \AG/NA\
and this implies that. h(A) = 1- ?
(b) Let G be an infinite cyclic group generated by g (say). Then P with
Pq = P\ = ZG, Pn = 0 for n > 2. di : P —>¦ Po the multiplication map by
3 — 1 and e : Pq —> Z the augmentation homomorphism is a G-projective
resolution of Z. Therefore for any left G-module A and any n > 2.
Hn(G,A)=Hn(G,A)=0.
We discuss the groups H°(G, A), Hl(G,A), Ho(G, A) and Hl (G, A) for
any group G in sections 3 and 4. So we do not discuss the case of particular
group here.
(c)
Lemma 11.2.3 // G is a group generated by a subset S of G, then the
augmentation ideal I(G) of the integral group ring ZG is generated as a left
G-module by the set {s — l\s G S}.
Proof. Observe that I(G) is generated as a Z -module by the set {x —
l\x G G}. Every element of G is of the form ns?; where Sj ? S and
every e, = 1 or —1. Since for x, y ? G, xy — 1 = x(y — 1) + (x — 1) and
x~l — 1 = —x~1(x - 1), I(G) is generated as a left G-module by the set
Theorem 11.2.4 Let F be a free group freely generated by a set of cardi-
cardinality at least two. Then I(F) is a free left ZF-module with \s — l\s ? S}
as a basis.
Proof. Let A be a left P-module and / : S - 1 = {s - l\s G S} -> A be
any map. Suppose that / can be extended to an P-homomorphism from
I(F) to A. From the above lemma it follows that such an extension is
necessarily unique. We now prove that the map / can be extended to an
P-homomorphism : I(F) —> A.
Consider the set A x F = {(«. x)\a ? A, x ? F} made into a group
under the composition (a,x)(b,y) = (a + xb,xy), a,b ? A,x,y ? G. Define
a map / : S -^_A x F by J(s) = (f(s -_l),s),s G S. Extend / to a
homomorphism / : F ^ Ax F. For x G F, f(x) can be uniquely written as
(ax,x). Define a map d:F-}Aby d(x) = ax,x G F where f(x) = (ax,x).
For s G S, (as,s) = f(s) = (f(s - 1), s) so that. as = f(s - 1). Since / is a
homomorphism. for x, y G F,
(axy,xy) =J(xy) =J(x)J(y) = (ax,x)(ay,y) = (ax +xay,xy).
© 2003 by CRC Press LLC
Therefore.
d(xy) = axy = ax + xay = d(x) + xd(y)
i.e. d is a derivation. Extend d by linearity to d* : ZF —> A i.e.
d*(^2miXi) = J^2rriid(xi), rrii ? Z. Xi ? F. Let d* also denote the re-
restriction of d* to I(F). For xt G F, rrn G Z.
d*C^2nii(xi - 1)) = d^^miXi-^mi) =d*(^2miXi)-d*(^2rrii)
Since d is a derivation. rf*(l) = d(l) = 0. Therefore
d*W_, mi(xi ~ 1)) = 5J rriidfai) = VJ rr
and for any x ? F.
d*(x'y2mi(xi ~ 1)) = rf* (y^j rrij (xxj - 1 - x
xd(xi))-
This proves that d* : I(F) —> A is an _F-homomorphism. For any s ?
S. d*(s — 1) = d(s) = as = f(s — 1) wihch proves that d* is an extension of
/. Hence I(F) is a free left _F-module with basis {s — l\s ? S}. q
Theorem 11.2.5 If F is a free group then Hn(F, A) = 0 = Hn(F, A) for
every left F- module A and for every n > 2.
Proof. Since 0 ->¦ I(F) ->¦ ZF ->¦ Z ->¦ 0 is an exact sequence and I(F)
is a free left (or right) -F-module. we get an _F-projective resolution P of
Z with Po = ZF, P, = I(F) and Pn = 0 for all n > 2. The result then
follows from the definition ofHn(F,A) and Hn(F,A). n
11.3 The Groups H°{G,A) and H0{G,A)
Let G be a group and A be a left G-module. Choose a projective resolution
P of the trivial G-module Z in which Po = ZG and e : Po ->¦ Z is the
augmentation homomorphism. Apply HorriG(—,A) to the exact sequence
^ -4- Z —> 0. We then get an exact sequence
0 ->¦ HomG(Z, A) 4 HomG{ZG, A) 5- HomG{P1, A).
© 2003 by CRC Press LLC
Therefore
H°(G,A) ^Kerd* = Ime* ^HomG(Z,A) 9= AG.
Next choose P as above but regarding Z as a right G-module under trivial
action. This means that every Pn will be a projective right G-module.
Apply the functor — ®G A to the exact sequence Pi —\- ZG -4- Z —> 0 to
get an exact sequence
Pi ®G A ^ ZG ®G A ^ Z ®G A -+ 0.
Then
H0(G,A) ^ ZG^oA/Imdi & 1 = Im (e ® 1) = Z ®G A ^ Z (
where K is the subgroup of ZtgiA generated by elements of the form n®a-
n (gi ga = n <g> A - ^)a. n ? Z. g ? G and nei. But Z 0 A = A under the
isomorphism n <g) a —>¦ na. n?Z. d?i Also under this isomorphism K
gets mapped onto {(g-l)a\a G /!} = /(G)^. Hence H0(G,A) ^ /1//(G)/1.
In case A is a trivial G-module; HG(G, A) ^ A ^ H0(G, A).
11.4 The Groups ^(G,^) and H^G.A).
Let G be any group and A be a G-module. Consider the exact sequence
0 —> I(G) A ZG -4- Z —> 0 where « is the inclusion map and e is the
augmentation map. Since ZG is G-free (both as left as well as right G-
module). the long exact sequences for homology/cohomology yield exact
sequences
>GAi^ ZG ®G A -> Z ®G A -* 0;
0 -^ iJomG(^,^) -^ HomG(ZG,A) ^ HomG(I(G),A)
Therefore.
HX{G,A) ^ Xer (z ® 1 : /(G) ®fi A ->
* : /(G) ®G A -* A)
where i*((g — 1) <8) a) = (g — l)a. g ? G. a ? A. and
H\G,A) ^ HomG(I(G),A)/Im(:HomG(ZG,A)^HomG(I(G),A))
~ HomG(I(G),A)/Im(i* : A -+ HomG(I(G),A)),
where i*(a)(g - 1) = (g - l)a, g ? G, a ? A. Recall that a map / : G ->¦ A
is called a derivation if f(xy) = f(x) + xf(y) for all x,y ? G. The set
© 2003 by CRC Press LLC
Der (G, A) of all derivations from G to A forms an Abelian group under the
usual addition namely, if /. g ? Der (G, A), then / + g : G —> A is defined
by (/ + g)(x) = f(x) + g(x). x ? G. A derivation / : G ->¦ A is called
an inner derivation or a principal crossed homomorphism if there
exists anaei such that. f(x) = (x — l)a. x ? G. The set Ider (G,A) of
all inner derivations from G to A forms a subgroup of Der (G, A).
Lemma 11.4.1 HamG(I(G),A) ^Der{G,A) and
Ira (i* :A^ HomG (I(G), 4)) = Ider (G,A).
Proof. For / G HamG(I(G),A): \e± J : G ^ A be. denned by
J(x) = f(x-1), x?G.
It is easily seen that. / is a derivation. Define a map 9 : Ho7tig(I(G), A) —>¦
?>er (G, i) by
O(f)=f; f?HomG(I(G),A).
The map 9 is clearly a homomorphism and since I(G) is generated by
x — 1. x ? G. 9 is & monomorphism. Let rf : G -> i be a derivation^
Extend of by linearity to d* : ZG —>¦ ^4 i.e. oT^miXi) = J]mjd(xj). Let d
be the restriction of d* to /(G). For x,y ? G.
d(x(y — 1)) = d(xy — x) = d(xy) — d(x) = d(x) + xd(y) — d(x)
= xd{y) = xd(y - 1)
which shows that, d is a G-homomorphism : I(G) —> A. Since d(l) = 0. it.
is clear from the definition of d that 9{d) = d. Hence 9 is an isomorphism.
Let a ? A. For x ? G,i*(a)(x) = i*(a)(x — 1) = (x — l)a. Therefore i* (a)
is an inner derivation : G —> A. If d : G —> A is an inner derivation, there
exists an a ? A such that d(x) = (x — l)a = i*(a)(x — 1) = i*(a)(x) which
shows that d = i*(a) = 9{i*{a)). This proves that 9(Imi*) = Ider (G,A)
and, so; Im (i* : A -> HomG{I{G),A)) ^ Ider (G, A). u
In view of the above lemma, we have
Proposition 11.4.2 Hl(G,A) ^ Der (G, A)/Ider (G, A).
Corollary 11.4.3 If A is a trivial G-module, then
Hl(G,A) ^
Proof. When A is a trivial G-module. (x — l)a = 0 for every x ?
G and a ? A. From this it follows that Ider (G, A) = 0. Also a map
d : G ->¦ A is a derivation if and only if d is a homomorphism. Let d
be a derivation from G to A. Then rf is a homomorphism and A being
an Abelian group d vanishes on the derived group G' of G. Therefore d
© 2003 by CRC Press LLC
induces a homomrphism d : G/G' —> A^d(xG') = d(x),x ? G. The map
(j>: Der (G, A) -> Hom(G/G', A) where <j){d) =d, d? Der (G, A) is easily
seen to be an isomorphism. Hence H1(G,A) = Hom(G/G',A). q
Proposition 11.4.4 If A is a trivial G-module , then
Hl{G,A)^(I(G)/I2(G))®A.
Proof. As proved earlier H1(GJ A) = Ker (z* : I(G) ®G A ->¦ A), where
z'*((x — 1) (8> a) = (a; — l)a,a; ? G,a ? A When ^4 is a trivial G-module.
(x — l)a = 0 for every x ? G and a ? A. Therefore, z* is the zero map and
iJi(G,^) ^ /(G) ®fi ^. The map I(G) xA^ (I(G) /12 (G)) ® A given by
(u, a) -^ (u + /2(G)) (g) a. u G /(G). nei
is biadditive and since A is a trivial G-module. it vanishes on elements
of the form (ux,a) - (u,xa), u G I(G), x G G. a? A Therefore, this
map induces a homomorphism a : I(G) ®g A —> G(G)//2(G)) 0 A where
a{u®a) = (u + I2(Gj) ®a. u G I(G). a ? A. The map a is obirously onto.
On the other hand, consider the map /3 : I(G) x A —> I(G) ®g A defined
by
0(u, a) =u<8>a. u? I(G). a ? A.
The map /3 is biadditive and for x,y ? G,a ? A.
P((x-l)(y-l),a) = (x - l)(y - 1) (i> a
= (x — l)y <8> a — (x — 1) <g) a
= (x — 1) <8> ya — (x — 1) <g) a
= (x - 1) (g) a - (x - 1) ® a = 0.
as ^4 is a trivial G-module. Therefore j3 defines a well defined biadditive
map P : G(G)//2(G)) x A -* I(G) (gia A given by
) =u®a., u? I(G), a ? A.
Thus 0 induces a homomorphism /? : (I(G)/I2(G)) ®A -^ 7(G)(g)G A That.
/Ja = identity map on 7(G) (g)c -4 and a/? = identity map on 7(G)/72(G) <&
^ are clear. Hence 7(G) ®G -4 = G(G)/72(G)) ® ^ and 77i(G,^) ^
G(G)/72(G))®AD
Lemma 11.4.5 G(G)/72(G)) ^ G/G'.
Proof. The map a : G/G' -> 7(G)/72(G) given by q(iG') = x - 1 +
72(G). x G G. is a well defined homomorphism (of Abelian groups). 7(G)
is a free Z-module freely generated by 5 = \x — l|x G G.x^ 1}. Extend
the map ? : 5 -> G/G' defined by
0(x-l) = xG': x? G, x^ 1
© 2003 by CRC Press LLC
to a Z-homomorphism ft : I(G) ->¦ G/G'. For x,y € G,
/3((x - l)(y - 1)) = p(xy - x - y + 1) = xyG''(xG'y^yG1)-1 = 1-
Therefore P induces a Z-homomorphism p : I(G)/I2(G) -> G/G'.
^ 2 (nx™;)G'; xf G G,m« G Z.
Both a/? and Pa are identity maps of the respective groups and. hence, a
is an isomorphism with P as it inverse, q
Combining Lemma 4.5 and Proposition 4.4. we get
Proposition 11.4.6 If A is a trivial G-module, them
i?i(G,A) =s (G/G') (8) A
We next consider an identification of H1 (G, .A) which is quite useful for
group theoretic applications.
Let H be any group and A be an Abelian normal subgroup of H. Let n :
FT —> H/A be the natural projection. Under conjugation by the elements of
H, A becomes an H/^-module. Let D be the set of all those automorphisms
a of H for which a(a) = a for every a ? A and n a = tt and Dq be the set
of all inner automorphisms of H which are defined by elements of A. Then
D is a group and -Do is a subgroup of D.
Theorem 11.4.7 The group H1 (H/'A, A) is isomorphic to D/Do-
Proof. Let a G D. Since 7r(a(/i)/i) = 1 for every h€ H, a^h'1 G A
Define a map o-' : H —> A by
() (): h?H.
For h, k?H,
a'(hk) = a(hk)k~1h-1 = a^h'1 {ha{k)k~l h~l) = a'(h)ha'(k)
showing that a' is a derivation. It is clear that a'(ha) = a'(h) for every
h G H. a G A and a' induces a map a" : H/A ->¦ A,
which is again a derivation.
Define a map 9 : D -> Der(H/A, A) by
8(a) = a"., a?D:
which is clearly one-one.
© 2003 by CRC Press LLC
On the other hand, let ft G Der(H/A, A) and define a : H ->¦ H by
a(h) = /3(hA)h, h G H.
For /i. ke H.ae A,
a(hk) = /3(hkA)hk
= /3(hA)(hP(kA)h~1)hk = a(h)a(k):
a(a) = /3(aA)a = a
and
a(h)A = p(hA)hA = hA., as p(hA) G A.
Thus a G D and it is clear that a" = j3. Hence 6 is onto as well.
Next, let a. /3 G D. For any he H,
(a/3)"(hA) = a/3{h)h-1 =a{/3{h))/3{h)-1f3{h)h-1
= a"(p(h)A)p"(hA) = a"(hA)p"(hA), as p(h)h~l G A
Therefore
Hence 9 is an isomorphism.
Let a G Inn (H) be determined by a ? A. Then
a"(hA) = a(h)h-1 =a(ha-1h-1) =a(/la) for every h G H.
Thus o-" G Ider (H/A, A) and is determined by a.
On the other hand, if ft G /der (H/A, A) determined by a ? A. then
a(/i) = p(hA)h = a(/la)/i = ft/m
and a G Inn (iJ) determined by a.
Hence under the isomorphism 9. the subgroup Dq of D gets mapped onto
the subgroup Ider (H/A, A) of Der (H/A, A). Therefore 9 induces an iso-
isomorphism :
D/Do ->¦ Der (H/A, A)/Ider (H/A, A) s* H1 (H/A, A). n
11.5 Homology and Cohomology of Direct
Sums
Let G be a group. R. be a ring and / : G ->¦ i? a multiplicative map with
/(e) = 1 where e denotes the idenitity of the group G. Then /* : ZG —> R
defined by f*(Y^mixi) = Y^rnif(xi) *s a homomorphism which extends /.
© 2003 by CRC Press LLC
If g : ZG —> R is another homomorphism which extends / i.e. g(x) = f(x)
for every x ? G. then for any element J] rriiXi ? ZG
Therefore. /* is the unique homomorphism which extends /.
We now prove that this property determines ZG.
Proposition 11.5.1 Let G be a group, R a ring and f : G —>¦ R a mul-
multiplicative map with f(e) = 1. If for any ring S and a muptiplicative map
g : G —>¦ S there is a unique homomorphism g* : R. —>¦ S such that g*f = g,
then R. = ZG.
Proof. By the property of the group ring proved above, there exists a
unique homomorphism /* : ZG —> R which extends /. Then /* \G= f. Let
i : G —> ZG denote the embedding of G in ZG so that i is multiplicative
and i(e) = 1. Then f*i = f. Also by the given property of R there exists
a homomorphism g* : R —>¦ ZG such that, g*f = i. Then (f*g*)f = f and
(9*' f*)i = i- By the uniqueness of the homomorphism in the given property
of R. and of the proven property of ZG we find that f*g* and g*f* are
identity maps of R. and ZG respectively. Hence /* is an ismorphism with
g* as its inverse. [—j
We are now in a position to describe group ring of a direct, sum of
groups.
Proposition 11.5.2 Let H, K be groups and G = H e K. Then ZG =
ZH&ZK.
Proof. Let R. S be two rings. In particular R. S are Z-modules and we
have an Abelian group R®S. For (r, s) ? Rx S. define a(r, s) : Z(R, S) —>¦
R <8 5 by
a(r, s)(r', s') - rr' <E> ss', (r', s') ? Rx S
and by linearity. The map a(r, s) vanishes on B(R, S) (cf 1.5.4 for notation)
and induces a homomorphism a(r, s) : Rg> S ->¦ i?<8 5. Thus, we get a map
a : RxS ->• Hom(R®S, R®S). Extend a by linearity to Z(R, S). The map
a is bilinear and it induces a homomorphism a : R®S ->¦ Horn(R®S, R<S>S).
For x,y ? R® S. define xy = a(x)(y). With this multiplication R& S
becomes a ring with 1 $5 1 as identity element. Observe that, for r,r' ?
R, 8,s' eS.,
(r (g) s)(r' <8> s') = a(r ® s)(r' <8> s') = (rr') $5 (ss').
Tn particular ZH (X> ZK is a ring with multiplication denned by
© 2003 by CRC Press LLC
where m^m'j G Z, ai,a!j G H and bt, b'j G K. The map / : H ® K ->•
Z77 ® /7/<f with f{h,k) = h <$S fc is clearly multiplicative and /A,1) =
identity of ZH" <? Z/f. Let R. be any ring and g : ff ® fT -> i? be a
multiplicative map with g(l,1) = 1. Define g* : ZH x ZK ->¦ i? by
^^ ^2inj9(ai,bj); a* ? H, bj ? K, rrii,nj G Z.
The map 3* is bilinear and induces an additive homomorphism 3* : ZH ®
ZK -»¦ i?. where for Oj ? H.bj ? K, m^rij G Z.
The map g being multiplicative, g* is a ring homomorphism. Also for
a? H. b? K.
which shows that g* f = g. Let a : ZH ® ZK —> B. be another homomor-
homomorphism such that af = g. For any element J] rriiai <8> bi G ZH ® ZK,
/\—^ \ \—^ / \ \—^
which proves that, a = g*. Hence g* : ZH $5 ZK —> R is the only homo-
homomorphism with g*f = g. Proposition 5.1 then shows that. ZG = ZH® ZK.
D
Proposition 11.5.3 Let H, K be groups, X be a free (left) H-m,od,ule and,
Y be a free (left) K-module. Then X ®Y is a free (left) G = H(B K-module.
Proof. Proceeding exactly as in the proof of Proposition 5.2. we can
prove that X <®Y becomes a ZG-modu\e under the scalar product defined
by
i ij
where nii ? Z. at ? H, bi ? K, Xj ? X. yj G Y. Since X and Y are
free H- respectively if-modules. X = C)Y,Ai, Y = ®Y,Bj, where every
At ^ ZH, and Bj ^ ZK. Now
where Cij = At <g> Bj = ZH ® ZK = ZG for every i, j. Hence X <g> Y is a
free ZG-module. q
Corollary 11.5.4 If P is a protective H-module and Q is a protective K-
module, then P ® Q is a projective G = H © K-module.
© 2003 by CRC Press LLC
Proof. Let X be a free iJ-module with a submodule P' such that. X =
P © P' and let Y be a free .ff-module with a submodule Q' such that
Y = Q © Q'. Then 1 ® 7 is a free G-module and
X ® Y = (P © P') ® (Q © 0') =s P g) Q © P g) 0' © P' ® Q © P' ® Q'.
Thus P & Q being a direct summand of a free G-module is a projective
G-module. q
Theorem 11.5.5 If G, G' are groups, (X, d), (X',d') are G-projective
respectively G' -projective resolutions of Z, then the complex X ® X' is G ©
G'-projective resolution of Z.
Proof. Since for n > 0. (X (g X')n = ©E^m ® X^_m and each
Xm (g X^_m is G © G'- projective module (Corollary 5.4). (X (g X')n is
a projective G © G'-module. The boundary operator Sn : (X $5 -X"')ra —>¦
(X <g) X')n_i on the generators is defined by
En(xm ® X^,_m) = dm(xm) (g) Xn_m + (-l)mXm (g) dt.-m^-m)
for 1 < to < n — 1 where xm ? Xm and x^_m G X'n_m and
Jra(x0 <S) xjj) = x0 (g) d^(x^) ftnrf <5ra(xra (g Xq) = dra(xn) (g Xq.
Observe that, then
Si (xi (g Xq + xo (g xi) = di (xi) (g Xq + x0 (g < (xi)
and also r? : Xo ® Jg —>¦ Z is defined by n(xo <8)x'o) = e(xo)e'(x'o). It is fairly
easy to see that. SnSn+i = 0 for n > 0. Also both e. f' being onto, for any
k ? Z we can find an xo G Xo and x0 G Xg such that e(xo) = fc. e'(x0) = 1
so that r?(xo ® x0) = fc. Thus r? is onto. (Observe that there is no disparity
with the definition of the boundary operator Sn defined on (X <g) X')n here
with the one defined in chapter 9 as there the complexes X, X' or Y do not
terminate in 0 on the right or left.) Each Xn is a ZG-projective module
and. so. is a direct, summand of a free ZG-module. Also every free ZG-
module being direct sum of copies of Z is a free Abelian group. Therefore
each Xn is a free Abelian group and. hence. Bn{X) = Zn(X) = Kerdn
is a free Abelian group. Moreover Hn(X) = Zn(X)/Bn(X) = 0 is also
projective Z-module. Therefore the Kw.nneth Tensor Formula (Theorem
9.3.2) is applicable and for every n, Hn(X ®X')^®Y, Hm(X) ® Hq(X').
However, for to > 1, q > 1, Hm(X) = 0; Hq(X') = 0 where as H0(X) ^
Z ^ H0(X'). Therefore Hn(X ® X') = 0 for n > 1 and H0(X ® X') ^ Z.
This proves that X®X' with the boundary operator S and n : X0<E>Xq ->¦ Z
as denned above is an exact sequence and. so. it. is a Z(G © G')-projective
resolution of Z. q
© 2003 by CRC Press LLC
11.5.6 Example
For an infinite cyclic group G generated by x (say), there is a G-free
resolution of Z of the form
?> 0 -?> ZG "-^ ZG 4 Z -s> 0
where x — 1 : ZG —> ZG denotes the multiplication by x — 1. Therefore, a
repeated application of the above theorem shows that, if G is a free Abelian
group of rank n, then there is a G-projective resolution P of Z with Pm = 0
for all m > n+1. Consequently Hm(G, A) = Hm{G, A) = 0 for all m > n+1
and all G-modules A.
We now consider a particular case of this : Let G be a free Abelian
group of rank 2 with u. v as free generators. Let H. K be infinite cyclic
groups generated by u. v respectively. Then we have exact sequences
o^zj?u41zff4x^ o.
0 -> ZK ZK 4 Z -> 0.
Combining these two we get a G-free resolution
s>O-s> ZH®ZK 4 (ZHig)ZK)®(ZHig)ZK) A ZH^ZK 4 Z -s> 0
where a,/? and 77 are defined as follows :
a(x ® y) = {(u — l)x ® y, —x $5 (w — l)y);
/J(x ® y, x' (g) y') = (u — l)x (g) y + x' $5 (w - I)?/',
r){x®y) = e{x)e'{y)
where x. x' G ZJJ and y. y' G Zif. Since ZG = ZH ig> ZK, we may take
the above resolution of Z in the form
A1.3) 0^ ZG ^> ZGS)ZGA ZG ^ Z ^0
where a. (i are defined by
a(x) = (ux, —vx). P(x,y) =vx + uy. u = u — 1. v = v — 1, x, y ? ZG.
That, a is a monomorphism. /3a = 0 and e/3 = 0 are clear. If /3(x, —y) =
0. then vx — uy = 0 i.e. (v — l)x — (u — l)y = 0 which implies that
x = (u — l)xi, y = (v — l)yi. Therefore, (u — l)(w — l)(xi — 2/1) = 0
which forces x\ = yi- Thus x = (u — l)x\. y = (v — l)xi and a(xi) =
((u - l)xi, —(v - l)xi) = (x, —jy). Therefore /ma = Ker 0. If e(x) = 0
for an x G ZG. then x = Xi(u — 1) + (w — l)yi for some Xi,yi G ZG and
© 2003 by CRC Press LLC
P(yi,xi) = x. This proves that, the sequence A1.3) is a G-free resolution
of Z. Tensor the sequence A1.3) with a G-module A over ZG. We get a
complex
where a. /3 are defined by
a(a) = ((u - l)a, -(v - l)a), ]3(a, b) = (v - l)a + (u - 1N. a. b G A.
Therefore H2(G,A) = Kera. For a trivial G-module A, a = 0 and. so.
Ker a = A. Hence, if A is any trivial G-module and G is free Abelian
group of rank 2. then i?2(G, A) = A. For cohomology we apply the functor
Ho7tig(—,A) to A1.3) and we get a complex
0 -> HomG (ZG,A)A HomG (ZG,A)® HomG (ZG,A)
4 HomG(ZG,A) -s>0
or
0 -^ A A A e A 4 A -+ 0
Observe that, a is obtained from a to make the following diagram commu-
commutative :
HomG(ZG®ZG,A)
HomG {ZG,A)@ HomG (ZG,A)
.40.4
a
HomG(ZG,A)
A
For / G HomG(ZG 8 ZG, A) we have the diagram
ta
© 2003 by CRC Press LLC
where f\. f'2 are restrictions of / to the frist and second component respec-
respectively. Now
(/a)(l) = /(a(l)) = f(u - 1, -(v - 1)) = (u - 1)/A) -(v- 1)/A).
Hence a : A © A -»¦ A is defined by 2<(a, b) = (u - l)a — (i> — 1N, a. 6 G A.
Then H2{G,A) = A/Ima = A/ < (u - l)a - (i> - lN|a, dVi > =
A/(I(G)A). In case A is a, trivial G-module. (u - l)a - (v - 1 )b = 0 for all
a., be A and, therefore, iJ2(G, A) ^ A
11.6 The Bar Resolution
Let G be a group. For n > 0. let Pn be the free G-module freely gen-
generated by the set of all ordered n-tuples [xi,X2, • • • ,xn], Xj G G. it being
understood that Po is the free G-module with a free G-basis consisting of a
singal element denoted by [ ]. For each n > 1. define a G-homomorphism
dn : Pn -> Pn-l by
d\ T1 ... np ^— IP i \ IPrt ••• T* 1
7i L 1) j **^ 71J — tlj\\_tlj 2 -j i J-jn\
where for n = 1, we mean d\ [x 1 ] = x 1 []-[]. Also define a G-homomorphism
e : Po —> Z, where Z is the additive group of integers regarded as a trivial G-
module, by e([ ]) = 1. On the other hand, let Fn be the free Z-module freely
generated by the set of all ordered n + 1-tuples < x0, x\, ¦ ¦ ¦, xn >. Xi G G.
For x, Xi, 0 < i < n, in G. define
X \ Xq j X\ j ' ' ' j Xji ^ — \ XXq j XX\ , " " " , XXji ^ -
With the G-action defined as above and linearity Fn becomes a G-module.
For each n > 1 define a Z-homomorphism 8n : Fn —>¦ Fn-i and So '¦ Fo —> Z
by
Sn < xo,xi,---,xn >= ^(-1)* < xo,---,Xj_i,fj,Xj+i,---,xra >;
<5n < xq >= 1; where'over an entry means that the particular entry is to be
omitted. Trivially 6n,n > 0. becomes a G-homomorphism. For each n > 1.
define a Z-homomorphism 6n : Fn —>¦ Pn by
"ra ^ 3^0i 3-li ¦ ¦ ¦ 1 Xn >= Xo[Xg Xi , X-y X2 , " ' " , Xn_^Xn\,
where Xj ? G. and define ^0 < xq >= xo[ ],xo ? G. Clearly every 6n
is a G-homomorphism. On the other hand, define for every n > 0. a G-
homomorphism <j>n : Pn —>¦ Fn by
4>n([xi, ¦ ¦ ¦ ,Xn]) =< l,Xl,XlX2, ¦ ' ¦ ,Xl • ¦ -Xn >,Xj G G.
© 2003 by CRC Press LLC
For Xi G G. 0 < i < n.
<t>n8n(< X0,Xl,-- -,Xn
and
= < Z0,Zl, ¦¦-,?„ >
¦ ¦ Xn
= xo[x1,x2,---,xn}.
Thus 8n(j)n and <f>n6n are identity maps of the G-modules Pn and Fn re-
respectively. Hence 8n : Fn ->¦ Pn and <f>n : Pn ^>- Fn axe G-isomorphisms.
Lemma 11.6.1 For oil n > 1. Jra_iJn = 0.
Proof. For xo,xi, ¦ ¦ ¦,xn+i € G. n > 0.
,Xi, • • • , Xi, ¦ ¦ ¦ , Xn+i
< X0,---,Xi,---,Xj,---,Xn+l >}
Lemma 11.6.2 Tftfi diagram
Pn+l
Fn+l
Sr
"n
Fn
>=0.
i+l
© 2003 by CRC Press LLC
is commutative.
Proof. For x\, ¦ ¦ ¦, xn+\ ? G.
= Sn+1 < l,Xl,XlX2, ¦ ' ¦ ,Xl • ¦ -Xn+i >
A1.4) = ^2(-iy < xo,xoxi, ¦ ¦ ¦ ,xoxi ¦ ¦ -Xi, ¦ ¦ ¦ ,xoxi ¦ ¦ -xn+i >
with Xo = 1. Also
= x^ <l,x2,x2x3,---,x2---xn+1>
,---,xi ¦¦¦xn+1
= < zi,zis;2, ¦ • ¦ ,xi ¦ ¦ -xn+i >
= ^2(-iy < X0,X0Xi, ¦ ¦ ¦ ,X0Xi ¦•¦'•¦ -Xi, ¦¦ • ,X0Xl ¦ ¦ -Xn+i >
= Sn+i<t>n+i[x1,- ¦ ¦ ,xn+1] (by A1.4)) n
Corollary 11.6.3 dndn+i = 0 for all n > 0, where do = ?•
Proof. For n > 1. consider the commutative diagram
p
n+l
"n
dn
Pn+1
^-l
Sn+1 Sn
in which the lower row is a O-sequence and the vertical maps are isomor-
isomorphisms. Then
4>n—l (dndn+l) = (SnSn+lJ&n+l = 0
which implies that. dndn+i = 0. Also, for any x ? G.
= x.l-1 = 0,
as e is a G-homomorphism and Z is a, trivial G-module. Thus ed\ = 0.
© 2003 by CRC Press LLC
Theorem 11.6.4 The sequence
A1.5) •¦¦->• Pn+1 H1 Pn dA Pn_! -> • • • -> P! ^ Po 4 Z -> 0
is a /ree ZG-resolution of the trivial G-module Z.
Proof. Corollary 6.3 shows that A1.5) is a O-sequence. To prove that
A1.5) is an exact sequence, observe that every Pn is a free Z-module with
{x\x\, ¦ ¦ ¦. xn] | x, Xi ? G} as a free Z-basis. Define Z-homomorphisms
sn:Pn^f Pn+1 (n > 0) by
sn(x[xi,---,xn]) = [x,x1,---,xn], x,xi,---,xn?G
and s-i : Z —> Po by s-i(l) = []• The homomorphisms sn and dn have
the following relations :
A1.6) es_i = 1. s_ie + dis0 = lp0. dn+isn + sn-idn = lpn. n > 1.
Since e([ ]) = 1, es_i(l) = 1 and. therefore. es_i = 1. For xi, • • • ,xn+\ ?
G,
(dn+isn + sn_1dn)(x1 [x2, ¦ ¦ -,xn+i])
= dn+i[xi,x2,- ¦ ¦ ,xn+i] + sn_i(xidn[x2,- • • ,xn+i])
= Xi[x2,- ¦ ¦ ,Xn+i] + ^2(-l)l[xi,- ¦ ¦ ,XiXi+i,- ¦ ¦ ,Xn+i]
2[x3. ¦••. xn+i]
—— nf-tlnfn ¦>¦ np -, —|— > I — 1 I T* i -¦- T* ¦ T* * i i -¦- Hf ii
Jb \ \Jb 2 j •}'*-' fl-\-\ J 1^ / \ / L -L' ~) 'L/ %'L/1~\- L •) ) "^ 77.-|-l J
+ ( 1 ^^"r-L \rtn ... rp 1 _L- r-7"-, rp ry ... ry 1
= Xi[x2)- • -,Xn+i] + ^(-l)*[xi,- ¦ ¦ ,XiXi+i,- ¦ ¦ ,Xn+i]
+ (-l)"+1[xi, • • • ,Xn] + ^(-l)*^!, ¦ ¦ -,XiXi+1,- ¦ -,Xn+1]
= xi[x2,---,xn].
Hence dn+\sn + sn_\dn is the identity map. The remaining relation may
be proved similarly. Now for a ? Kerdn. n > 1. we have
a = (dn+\sn + sn-\dn)(d) = dn+i(sn(a)) G Imdn+i.
Thus, for every n > 1. Kerdn = Imdn+i.
Again, for a G Ker e we have
a = (s_ie + diso)(a) = di(so(a))
© 2003 by CRC Press LLC
which is in the image of d\. Therefore. Kere = Imd\. That, e is an
epimorphism follows from the first relation in A1.6). This proves that the
sequence A1.5) is exact. Hence A1-5) is a ZG-fie.e. resolution of Z. q
The ZG-free resolution A1-5) is called the Bar Resolution of Z.
11.6.5 Cochains
Let A be a G-module. For n > 0. let Cn(G,A) denote the set of all
maps/:Gx---xG-> A n> 1, with C°(G, A) = A. For f,g G Cn(G, A),
define f + g: Gx---xG^-A by
(f + d)(xi,---,Xn) = f(xi,---,Xn)+g(xi,---,Xn), Xi G G.
This composition makes Cn(G,A) into an Abelian group. On the other
hand, we also have an Abelian group HomG(Pn,A). Define maps
6n : Cn{G,A) -> HomG(Pn,A): <j>n : HomG{Pn,A) -> Cn(G,A)
by
6n(f) = f,f?Cn(G,A)
and
4>n(g) = a, 9 G HomG(Pn, A)
where
J(^2zi[x1,---,xn]) = '^2zif(x1,---,xn), Zi,Xj G G.
g(xi,---,xn) =g([x1,---,xn\), xt G G.
The maps 8n and 4>n are group homomorphisms with <j>n6n and 0n<t>n iden-
identity maps of Cn(G,A) and Hom,G(Pn,A) respectively. Thus the groups
Cn(G, A) and HomG(Pn, A) are isomorphic. For n > 0, define d" : C"(G, A)
It is fairly easy to see that, the diagram
¦ HomG(Pn-i,A)
© 2003 by CRC Press LLC
is commutative. Therefore the two complexes have isomorphic homologies.
i.e., Hn(G,A) ^ Kerdn/Imdn-1.
The elements of Cn(G,A) are called n-cochains. those of Kerd" de-
denoted also by Zn(G, A) are called n-cocycles of G with coefficients is A and
those of Im d,n~1 also denoted by Bn(G,A) axe called n-coboundaries.
For n = l, Cn~l{G, A) = C°{G, A) = A and for any a ? A, d°(a) = adi =
/ (say) such that f(x) = xa — a. x G G. Thus
B°(G,A) = {/ G C1(G, A)\there exists an a G A with f (x) = xa - a} .
Also
Z°(G, A) = {a G A | d° (a) = Sdi = 0}
= {a G A\xa - a = 0 , /or a// x G G} = AG.
Definition 11.6.6 An n-r.or.hain f G Cn(G,A) is said to be normalized
if f(xi, ¦ ¦ ¦ ,xn) = 0 whenever any one of x\, ¦ ¦ ¦ ,xn is the identity elem.ent
ofG.
Let C'n(G,A) denote the set of all normalized n-cochains of G with
coefficients in A. Z'n(G,A) be the set of all normalized n-cocycles. Then
C'n{G, A) is a subgroup of Cn(G, A) and Z'n(G, A) is a subgroup of Zn(G, A).
Let B'n(G,A) be the set of all n-coboundaries dn~lf., f G C'n~l{G,A).
Then B'n(G, A) is a subgroup of Zn(G, A). Also it is clear from the defini-
definition of the coboundary operator dn that. dnf is normalized if / is normal-
normalized. Therefore B'n(G,A) is a subgroup of Z'n(G,A).
Let H'n(G,A) = Z'n(G,A)/B'n(G,A). The obvious inclusion maps
induce a homomorphism : H'n(G,A) —> Hn{G,A). This homomorphism is
indeed an isomorphism and follows from the lemmas
Lemma 11.6.7 Every cocycle is cohologous to a normalized cocycle. (Two
cochains which differ by a coboundary are called cohomologous.)
Lemma 11.6.8 Every normalized, enchain which is a coboundary is the
coboundary of a normalized cochain.
Tn view of the above observation while studying cohomology groups of
a group G with coefficients in a G-module. we can always consider only
normalized cochains.
11.6.9 Exercises
1. Let G be a free Abelian group with {u. v} as a basis. If x. y G ZG
such that (v — l)x — (u — V)y = 0. show that x = (u — l)x\. y = (v — 1J/1,
for some x\. y\ G ZG. Also prove that if (u-l)(v - l)x = 0 for an x G ZG.
then x = 0.
2. Prove Lemmas 6.7 and 6.8
© 2003 by CRC Press LLC
11.7 Second Cohomology Group and Exten-
Extensions
By a group extension of an Abelian Group A by a group G we mean an
exact sequence
A1.7) B:0444M4G4l
of groups. By identifying A with its image i(A) in M we may regard A as
a subgroup of M and i the inclusion map. Two extensions
and
are said to be equivalent if there exists a homomorphism a : Mi —> Mi
inducing the identity maps on A and G i.e. the diagram
a ||
A1.8) " " 2 " ' *
is commutative. Observe that a will necessarily be an isomorphism.
Let a group extension E as in A1.7) be given. For every x G G, choose
an element u(x) ? M such that n(u(x)) = x. Then {u(x)}xeG is called a set
of representatives of the elements of G in M. Tf {v(x)}xeG. is another set
of representatives of the elements of G in M. then n(u(x)) = x = n(v(x))
for every x ? G and there exist elements a(x) ? A such that v(x) =
a(x)u(x). x ? G.
For x G G, a ? A,
ir(u(x)au(x)~1) = ir{u{x))iT{a)iT{u{x))~l = xlx~l = 1.
so thai u(x)au(x)~l G A and
v(x)av(x)~1 = a(x)(u(x)au(x)~1)a(x)~1 = u(x)au(x)~1.
as A is Abelian. Thus. u{x)au(x)~l is independent of the choice of repre-
representatives of the elements of G in M. We define
xa = u(x)au(x)~1 ,x G G. a G A.
For x, y G G, a G A,
x(ya) = x(u(y)au(y)~l)
A1.9) = u(x)(u(y)au(y)-1)u(x)-1 = {u{x)u{y))a{u{x)u{y))-1.
© 2003 by CRC Press LLC
Also
n(u(x)u(y)) = n(u(x))n(u(y)) = xy = n(u(xy)).
Therefore, there exists an element f(x,y) ? A such that
u(x)u(y) = f(x,y)u(xy).
Using this relation in A1.9) we get
x(ya) = f(x,y)(u(xy)au(xy)~1)f(x,y)~1 = u(xy)au(xy)~1 = (xy)a.
Observe that, for the identity 1 of G. u(l) ? A and so
l.a = u{l)au{l)~l = a far every a ? A.
That. x(a + b) = xa + xb, x ? G, a,b ? A also follows easily. Hence A
becomes a G-module. This module structure on A is called the module
structure induced by the extension R.
Let E-\ and E% be two equivalent extensions of A by G. Then we have
a commutative diagram A1.8). Let {ui(x)}xeG be a set of representatives
of the elements of G in Mi. Then
TT2(aui(x)) = ni(ui(x)) = x for every x ? G.
Hence. {u2 (x)}xeG- where U2(x) = a{u\{x)). x ? G is a set of representa-
representatives of the elements of G in Mi and for x ? G. a ? A.
U2(x)au2(x)~1 = aui(x)aaui(x)~1
= a{u\{x)aui{x)~l). as a(b) = b for b ? A:
= ui(x)aui(x)~1.
Thus, equivalent extensions induce the same G-module structure on A.
Suppose that A already carries a given G-module structure. We restrict
attention to the set S of only those extensions of A by G which induce
the given G-module structure on A. The relation of two extensions being
'equivalent' is an equivalence relation and partitions the set 5 into equiva-
equivalence classes. We denote this set of equivalence classes of extensions of A
by Gby opext(G,A).
Theorem 11.7.1 There is a one-to-one correspondence between the ele-
elements of opext{G,A) and those of H2(G,A).
Proof. Consider an extension E of A by G as in A1.7) and let {u(x)}xeG
be a set of representatives of the elements of G in M. While proving that.
© 2003 by CRC Press LLC
E induces a G-module structure on A. we proved that, for every x. y ? G.
there exists a uniquely determined element f(x,y) ? A such that
u(x)u(y) = f(x,y)u(xy).
For x.y.zG G.
A1.10) u(x)(u(y)u(z)) = u(x)f(y,z)u(yz)
= u(x)f(y,z)u(x)~1u(x)u(yz)
= (xf(y,z))f(x,yz)u(xyz)
and
A1.11) (u(x)u(y))u(z) = (f(x,y)u(xy))u(z)
= f(x,y)u(xy)u(z)
= f(x,y)f(xy,z)u(xyz).
Multiplication in M being associative, it. follows from A1.10) and A1.11)
thai
xf(y, z) + f(x, yz) = f(x, y) + f(xy, z)
or
xf(y, z) - f(xy, z) + f(x, yz) - f(x, y) = 0
showing that / is a 2-cocycle.
(Watch! We are using additive composition in A but multiplicative
composition in M and as such the relation between elements of A that we
obtain from A1.10) and A1.11) has been changed into additive notation.)
Observe that by restricting the representatives {u(x)}xGG to satisfy
u(l) = 1. we make the corresponding 2-cocycle / normalized. We assume
this restriction henceforth.
Let {v(x)}X?G be another set of representatives of the elements of G in
M and let g : G x G —> A be the 2-cocycle corresponding to this choice of
representatives. Then tt(u(x)) = x = n(v(x)) for every x ? G. and so there
exist, elements a(x) ? A such that v(x) = a(x)u(x). x ? G. For x. y ? G.
g(x,y)v(xy) = v(x)v(y)
= a(x)u(x)a(y)u(y)
= a(x)u(x)a(y)u(x)~1u(x)u(y)
= a(x)(xa(y))f(x,y)u(xy)
= a(x)(xa(y))f(x,y)a(xy)~1v(xy).
Therefore.
g(x, y) = a(x) + xa(y) - a(xy) + f(x, y)
so that / and g differ by a 2-coboundary. Hence / + B2(G, A) G H2(G, A)
does not depend on the choice of the representatives {u(x)}xGG-
© 2003 by CRC Press LLC
Let E' : 0 -»¦ A ->¦ M' ^> G -»¦ 1 be an extension equivalent to _B with
a : Af —>¦ Af' a homomorphism inducing the identity maps on A and G. We
can choose a set of representatives {v(x)}x^g of the elements of G in M'
by setting v(x) = au(x), x ? G. The 2-cocycle associated with this choice
of representatives is clearly / again. Thus equivalent extensions yield the
same element, of H2(G, A). We define a map
6:opext(G,A) ^H2(G,A)
by setting 0([E]) = f + B2(G, A), where [E] denotes the class of extensions
of A by G which axe equivalent to E and / is a 2-cocycle as obtained
above. Suppose that 6{[E]) = 0([E']), where E is as in A1.7) and E' is
Q-tA-tM'^G-tl. Let / : G x G -»¦ A be the 2-cocycle associated
with the choice \u(x)}x^g of representatives for E and g : G x G —> A be
the 2-cocycle associated with the choice \v(x)} of representatives for E'.
Then there exists a tp ? G1(G, A) such that g = f — d}ip. Every element
of M can be uniquely written as au(x), a ? A, x ? G and every element
of M' can be uniquely written as av(x), a ? A, x ? G . Define a map
a : M -> M' by
a(au(x)) = aip(x)v(x), a ? A, x ? G.
Then, for x. y?G. a.b?A,
a(au(x)bu(y)) = a(au(x)bu(x)~1 f(x,y)u(xy))
= a(xb)f(x,y)ip(xy)v(xy).
On the other hand.
a(au(x))a(bu{y))
= aip(x)v(x)bip(y)v(y)
= aip(x)(v(x)bv(x)~1)(v(x)ip(y)v(x)~1)g(x,y)v(xy)
= aip(x)(xb)(xip(y))f(x, y)((xi(;(y))i(;(xy)~1i(;(x))~1v(xy)
= a(xb)ip(xy)f(x,y)v(xy)
= a(au(x)bu(y)).
Thus, a is a homomorphism.
It is clear from the definition of a that it induces the identity map on A
and on G. Hence E and E' axe equivalent and 9 is one-one.
Conversely suppose that f : G xG —$¦ A is a 2-cocycle. which, in view of
Lemma 6.7, we may assume is normalized. Let M = {(a,x)\a ? A. x ? G}
and define
(a,x)(b,y) = (a + xb + f(x, y),xy). a. b ? A. x. y ? G.
© 2003 by CRC Press LLC
Using the 2-cocycle property of / it is easily checked that multiplication in
M is associative. Also since / is normalized.
@,1) (a, x) = (a, x) = (a, x) @,1) for all (a, x) G M.
For x GG,
x~1f(x,x~1) - f{x~lx,x~l) + f{x~l,xx~l) - f{x~l,x) =0.
Therefore, again using / is normalized.
x-1f(x,x-1) = f(x-\x)
Hence, for x G G. a G A,
(—x~1a — x~x f (x, x~x), x~1)(a, x)
= (—x~la — x~l f(x,x~l) + x~la + f(x~1,x),x~1x)
= (-x-1f(x,x-1)+f(x-1,x),l)
= @,1)
and
(a, x){—x~x a — x~1/(x,x~1),x~1)
= (a — xx~la — xx~l f(x,x~1) + f(x,x~1), 1)
= @,1)
Thus. M is a group. The maps i : A ^ M, n : M ^ G denned by
i(a) = (a, 1). ir(a,x) = x, a G A, x G G
are homomorphisms and the sequence
B:0444M4G-> 1
is exact. For x G G, choose u(x) = @,x). Then
u{x)i{a)u{x)-1 = @,i)(o,1)(-i-1/(i,i-1),i-1)
= (xa + f(x, l),x)(-x~1f(x, x-l),x~l)
= (xa,x)(—x~1f(x,x~1),x~1)
= (xa — xx~Y f(x,x~1) + f(x,x~1), 1)
= (xa, 1) = i(xa).
Thus the extension E induces the given G-module structure on A. Also.
for x, y G G.
u(x)u(y) = @,x)@,y) = (f(x,y),xy) = (f(x,y),l)@,xy) = i(f(x,y))u(xy).
The above choice of representatives thus give the 2-cocycle / and hence
6([E]) = f + B2(G,A). The map 6 is thus onto as well. Q
© 2003 by CRC Press LLC
11.7.2 Exercises
1. If G is an infinite cyclic group and A is any G-module. prove that
every extension of A by G splits. Is the result true if (i) G is a free Abelian
group of finite rank > 2 ? or (ii) G is a finite cyclic group ? Justify in each
case.
2. If F is a free group and A is any F-module, prove that every extension
of A by F splits.
3. If G is a finite group of order rn. A is a finite G-module of order n
and g.c.d(m,n) = 1. prove that every extension of A by G splits.
11.8 Some Homomorphisms
Let G be a group and H be a subgroup of G. We have proved that the
integral group ring ZG is a free left ZH-module.. Let P be a projective left
Z G-module. Then there exists a free left ZG-module F and a submodule Q
of F such that F = P Q)Q. Now F being free is direct sum of copies of ZG
and ZG is direct sum of copies of ZH. Therefore F is direct sum of copies
of ZH and F is a free ZH-module. As P and Q can both be regarded as
ZH- modules, the direct summand P of F is a projective ZH-module. We
have thus proved
Proposition 11.8.1 Every projective ZG-module is a projective ZH-module.
The corresponding result for injective modules is also true but the proof
is not that simple. Although we have no immediate use of this result, we
prove it here for completeness.
Theorem 11.8.2 For left H-module A and left G-module B, there is a
natural equivalence : ExtG{ZG®H A,B) -> ExtnH(A,B) for all n > 0.
Proof. It follows from Theorem 3.4.5 that there is a canonical isomor-
isomorphism : HomG(ZG ®H A, B) -> HomH{A, HomG(ZG, B)). Also HomG
(ZG, B) = B canonically. Therefore, there is a canonical isomorphism a :
HomG(ZG (E>h A,B) -> HomH(A,B). Now ZG (E>H ZH ^ ZG as left
ZG-modules and it can be used to prove that if P is a Zif-projective mod-
module, then ZG ®h P is a ZG-projective module. Choose a zJiJ-projective
resolution
P: >
of A. Tensoring this with ZG over ZH. we get an exact sequence
ZG ®H Pa -> ZG ®H A -> 0
© 2003 by CRC Press LLC
in which every ZG ®h Pn is ZG-projective. Thus ZG ®h P is a ZG-
projective resolution of ZG®h A. Applying HorriH (—,B) a,nd Homo (—, B)
to the deleted complexes of P and ZG ® h P and making use of the natural
isomorphism a. we get a commutative diagram
HomG(ZG(E>HPo,B) - ¦¦¦ - HomG(ZG (g>H Pn,B)
HomH(P0,B) - ••• HomH(Pn,B)
HomG(ZG(E>HPn+i,B) - •••
* HomH(Pn+i,B) - ¦¦¦
with each vertical map a canonical isomorphism. Taking homology we get
canonical ismorphisms
Extn(ZG ®h A, B) ^ Ext%(A, B) for all n > 0. Q
Theorem 11.8.3 A left R-module E is injective if and only if for every
left ideal J of R, ExtlR(R/J,E) = 0.
Proof. If E is an injective _ff-module,then Ext^(A, E) = 0 for every left
i?-module A and all n > 1. In particular. Ext1R(R/J,E) = 0 for every left
ideal J of B.
Conversely, suppose that Ext^R/'J,E) = 0 for every left ideal J of
B. Let A be a submodule of B and / : A —> E be an B- homomorphism.
Consider all pairs (C, g) such that C is a submodule of B containing A and
g : C —> E is an extension of / : A —> E. Partially order this set of all
pairs by saying that (C, g) < (C',g') if C C C" and #' is an extension of 3.
Tf {(Ci,gi)} is a linearly ordered set of such pairs, we take C = UCi and
define h : C —> E by h(c) = <?i(c) when c ? Ci. Then G is a submodule of B
with A C C and ft, is an _R-homomorphism which extends /. Also, clearly
(Cj, <7j, ) < (C, h) for every ordered pair (Cj, #») in the linearly ordered set.
Therefore, by Zorn's lemma there is a maximal such pair (D,g) say. We
claim that D = B. Tf not. there exists an element b ? B. b <? D . Let
D' = D + Rb i.e. D' is the submodule of B generated by D and b. Let J
be the kernel of the epimorphism a : B. -»¦ D'/D, a(r) = rb + D, r G B.
Thus R/J = D'/D. Initial part of the long exact sequence for cohomology
corresponding to the exact sequence 0 —> D —> D' —> D' /D —> 0 gives an
© 2003 by CRC Press LLC
exact sequence
HomR{D',E) -> HomR{D,E) -> ExtR(D'/D,E) Sa ExtR{R/J,E) = 0.
Therefore Hom,R(D',E) ->¦ Hom,R(D,E) is an epimorphism. In particular
G can be extended to a homomorphism g' : D' —>¦ E showing that (D,g) <
(D',g') which contradicts the choice of (D,g). Hence D = B and g extends
/ : A —> E to B. Therefore E is an injective -ff-module. q
Theorem 11.8.4 Every G-injective module is H-injective.
Proof. Let E be an injective G-module. Let J be a left ideal of ZH.
Then, for every n > 1 (Theorem 8.2).
ExtnH(ZH/J, E) ^ ExtG(ZG ®H ZH/J, E) = 0.
It then follows from Theorem 8.3 that E is injective as an H-module. q
We now return to our main theme.
Let A be a left G-module and B a right G-module. Choose a projective
ZG-resolution
P : > Pn+1 ~^Pn^ >Po^ Z ^ 0
of the trivial left G-module Z. Then P is also a ZiJ-projective resolu-
resolution of Z. For left G-modules X. Y and right G-module X'. every G-
homomorphism : X —> Y is an _ff-homomorphism and X' ®G Y is homo-
morphic image of X' <%>h Y. We. therefore, have commutative diagrams
HomG(P0,A)-
A1.12)
and
ra+l-
B ®H Pn "¦ "¦ B ®H Po *¦ 0
¦¦¦ ^8GFn+1^ B®GPn —* .S®GPO^ 0
A1.13)
On taking homology. the above diagrams induce homomorphisms
res:H"(G,A) -> Hn(H,A):
© 2003 by CRC Press LLC
cores : Hn(H,B) -> Hn(G,B)
which are respectively called restriction and corestriction homomor-
homomorphism. We could equally well take P to be the bax resolution. Then the
restriction homomorphism is defined as follows : If f : G x ¦ ¦ ¦ x G —> A is
an n-cocycle. then / restricted to FT x ¦ ¦ ¦ x FT is also an n-cocycle / (say)
and res ([/]) = [/]. where [g] denotes the element of Hn(—,A) defined by
the n-cocycle g.
Lemma 11.8.5 Let H be a subgroup of finite index in a group G. Then
there exists a natural transformation of bifunctors from Hoi71h(—,—) to
Hoitig(—,—); the functors being defined : Q x Q —»¦ Ab where Q is the
category of G-modules and Ab is the category of Abelian groups.
Proof. Let [G : H] = k < oc and T = {tt | 1 < i < k} be a left
transversal of FT in G. Let X and A be G-modules. Then these are FT-
modules as well. For / G Hoitih(X,A),x ? X. define
= Z1<i<ktif(t-1x).
If {si | 1 < i < k} is another left transversal of H in G. then Si =
for some hi G H,l <i < k. For x ? X.
Thus 9(f)(x) is independent of the choice of the left transversal T of H in
G. That 6(f)(x + y) = 6(f)(x) + 8(f)(y) for all x. y G X is easy to see.
Also, when g G G. we have
0(f)(gx) = VUf{t l
Now g~lti = Ujhj for some tij G T. hj G FT. Therefore.
e(f)(gx) = SUfihjhJx) = SUhjifitJx)
= gZtijf(t-j1x)=g6(f)(x).
Thus 9(f) G Homa{X, A) and we get a map
6 : HomH{X,A) -> HomG(X,A).
The map 6 is clearly a group homomorphism.
Let X. Y. A. B be G-modules and a : Y -> X. /3 : A -> B be G-
homomorphisms. For / G Hoitih(X,A). y ?Y.
((Hom(a,p)e)(f)(y) = (Ham(a,0)F(f)))y
= (pe(f)a)(y)
= 0(Pfa)(y)
= (FHom(a,p))(f))(y)-
Therefore. Hom(a,f3N = 6Hom(a,P) proving that the diagram
© 2003 by CRC Press LLC
HomH{X,A)
Hom(a,P)
HomH(Y,B)
HomG(X,A)
Hom(a,/3)
HomG(Y,B)
is commutative. This proves that 6 : Homni-,-) ~^ HomG(-,-) is a
natural transformation of bifunctors. q
Theorem 11.8.6 Let H be a subgroup of finite index k in a group G. Then
for every n > 0 and G-m,odule A, there exists a group homomorphism Cor :
Hn(H,A) -> Hn(G,A) such that Cor o res : Hn(G,A) -> Hn(G,A) is
multiplication by k.
Proof. Let X be a G-module and 6 : Homn(X,A) -»¦ HomG(X,A)
be the homomorphism as defined in the proof of Lemma 8.5. Every / ?
HomG [X, A) can be regarded as an H-homomorphism and we write / =
res (/) for / when regarded as an H-homomorphism. For / ? HomG(X, A).
xGX.
0(f)(x) =
= kf(x): as f is a G — homomorphism
= (kf)(x).
Hence 6{f) = kf which implies that 6 ores: HomG(X, A) ->¦ HomG(X, A)
is multiplication by k. Let
be the standard bar resolution of Z over G. Then every Ft is a free G-
module and. hence, a free H-module. Applying the functors HomG(-,A)
and HomH(—, A) to the bar resolution and using the fact that 9 is a natural
transformation, we get a commutative diagram
HomH(F0,A)
0 HomG(F0,A)
HomH{Fn,A)
HomG(Fn,A)
HomH(FuA)
HomG(FuA)
HomH(Fn+i,A)
HomG(Fn+1,A)
© 2003 by CRC Press LLC
The homomorphisms 6 then induce homomorphisms on the homology groups
Cor : Hn(H, A) -> Hn(G, A) defined by
Cor (/ + Bn(H, A)) = 6(f) + Bn(G, A)., f G Zn(H,A)(= Kerd*n+1).
Let / G Ker(d*n+1 : HomG(Fn,A) -> HomG(Fn+1,A)). Then
(Corores)(f + Bn(G,A))
= 6 (res (/)) + Bn(G, A) = kf + Bn(G, A)
= k(f + Bn(G,A)).D
Corollary 11.8.7 // G is a finite group of order m and A is any G-module,
then m Hn(G, A) = 0 for all n > 1.
Proof. Taking FT = {1} we find that the composition map
Hn(G, A) r4s Hn(H, A) C4r Hn(G, A)
is multiplication by m. But Hn(H, A) = 0 for all n > 1. Hence
mHn(G, A) = 0 for all n > 1. n
Corollary 11.8.8 Let G be a finite group and Gp be a sylow p-subgroup of
G, p a prime. Then for every G-module A and n > 1, the sylow p-subgroup
Hn(G,A)p ofHn(G,A) is isomorphic to a subgroup ofHn(Gp,A).
Proof. Let o(G) = mpk where p does not divide m. Then [G : Gp] = m.
Consider the restriction of the homomorphism
res : Hn{G,A) -> Hn(Gp,A), n>l,
to the subgroup Hn(G, A)p. Let ? G Hn(G, A)p such that res (^) = 0. Then
m^ = 0. But o(?) = pr for some non-negative integer r and (m,pr) = 1. It
follows that ? = 0. This proves that
res : Hn(G,A)p -> Hn(Gp,A), n > 1
is a monomorphism. [—j
Corollary 11.8.9 Lei G be a finite group. Then, for every G-module A
and n > 1. Hn(G, A) is isomorphic to a subgroup of(S)Yl Hn(Gp, A), where
Gp denotes a sylow p-subgroup of G and p \ o(G).
Using Corollary 8.7 we can now prove that for a finite group G all Hn(G, A),
n > 1. for a suitable A are finite groups. First we have
Theorem 11.8.10 // G is a finite group and A is a torsion free divisible
Abelian group regarded as a G-module in any manner, then Hn(G,A) = 0
for all n > 1.
© 2003 by CRC Press LLC
Proof. Let m be the order of G. Let n > 1 and f:GxGx---xG^>-A
be an n-cocycle. Then mf is a coboundary. say Sg, where g is an n — 1
cochain from G to A. Since A is a torsion free divisible Abelian group,
there exists an n — 1 cochain h : G x • • • x G —> A such that g = rah.
Therefore mf = Sg = S(mh) = m.Sh giving m(f — Sh) = 0. The group A
being torsion free, we get / — S h = 0. This proves that / is a coboundary.
Hence Hn(G,A) = 0. Q
Proposition 11.8.11 // G is a finite group and G-module A is finitely
generated as an Abelian group, then Hn(G, A) is finite for all n > 1.
Proof. Let \G\ = m (< oo). Then mHn(G,A) = 0 for all n > 1 i.e.
Hn(G, A) is a tortion group.
Let Pn(G) denote the nth term in the standard bar resolution of Z.
Pn(G) is a free ZG-module of finite rank and. therefore. HomG{Pn{G),A)
is isomorphic to direct sum of a finite number of copies of A. Therefore
HomG(Pn(G),A) is finitely generated. Hence, for n > 1. Hn(G,A) is a
finitely generated torsion group and is. therefore, finite, q
Corollary 11.8.12 // G is a finite group and G-module A is a finite p-
group, then Hn(G, A) is a finite p-group for all n > 1.
Theorem 11.8.13 Let G be a finite group, Q the additive group of ratio-
nals and T = Q/Z, the additive group of rationals mod 1 regarded as a
trivial G-m,odule. Then for every n > 1. Hn(G,T) is a finite group.
Proof. Let n > 1. The long exact sequence for cohomology correspond-
corresponding to the exact sequence 0—> Z —> Q —> T —> 0 gives an exact sequence
Hn(G,Q) -> Hn(G,T) -> Hn+1(G,Z) -> Hn+1(G,Q).
By the theorem above Hn(G, Q) = Hn+1 (G, Q) = 0. Therefore Hn(G, T) ^
Hn+1(G,Z). By Proposition 8.11, Hn+1(G,Z) is finite. Hence Hn(G,T)
is finite for all n > I.q
Suppose now that FT is a normal subgroup of G.
Lemma 11.8.14 If A, B are left G-modules, then HomH(A,B) is a G/H-
module.
Proof. For x G G. f G HomH(A,B): define xf : A -> B by
(xf)(a) = xf(x~1a),a G A.
For a G A, he H.
(xf)(ha) = xf{x-lha) = xf{x-lhxx-la) = hxf{X-la) = h(xf)(a).
© 2003 by CRC Press LLC
Also xf is clearly additive. Therefore xf ? Hoi71h(A,B). Again, for
h?H.a?A. '
(hf)(a) = hfih-'a) = f(a)
showing that elements of H operate trivially on HomniA, B). This gives a
well defined action oiG/H on Hoitih(A, B) as follows : For / G Hoitih(A, B).
xgG.,
(xH.f)(a) = xf(x~1a). a ? A.
With this action Hoitih(A,B) does indeed become a G/H-module. q
Let A be a left G-module. Choose a protective ZG-resolution P of the
trivial G-module Z. Then P is also a ZiJ-projective resolution of Z and
Hn(H,A) = H(HomH(Pn-i,A) - HomH(Pn,A) - HomH(Pn+i,A)).
Let /. g : Pn —> A be two cohomologous n-cocycles. Then there exists a
A G Hom,H(Pn-i,A) such that g = f + \dn. For x G G. define fj,x : Pn-\ -»¦
A by
fj,x(b) =x\{x~1b),b G Pn-i.
For b G Pn-i, h? H,
Hx(hb) = x\(x~1hb) = x\(x~1hxx~1b)
= hx\(x~1b) = h(j,x(b)
which shows that /ix is an H-homomorphism. Now. for b G Pn-
(xg)(b) = xg{x~lb) = xf{x~lb) + x{\dn){x-lb)
= {xf){b)+x\{x-ldn{b))
= (xf)(b) + nx(dn(b)) = (xf + nxdn)(b).
Thus xg = xf + [ixdn showing that xg and x f differ by a coboundary.
We can. therefore, define x[f] = [x f], giving a well defined action of G on
Hn(H,A). With this action Hn(H,A) becomes a G-module.
Lemma 11.8.15 When H is normal in G, the image of res : Hn(G,A) —>¦
Hn(H,A) lies in Hn(H,A)G.
Proof. Choose a projective ZG-resolution P of A and consider the com-
commutative diagram A1.12). Let / G HomG(Pn-,A) be an n-cocycle. Then
res([f]) = [/]. where / is the map / regarded as an H-homomorphism.
For x G G. a G Pn.
(xj)(a) = xJix-U) = xfix-U) = f(a) = /(a).
Therefore xf = J and x[J] = [xj] = [/] showing that [/] G Hn(H, A)G. Q
Next two results are good examples on applications of Theorem 7.1.
© 2003 by CRC Press LLC
Proposition 11.8.16 If D is a divisible Abelian group regarded as a trivial
G-module and the subgroup H of G is central and is also contained in the
derived group G' of G, then res : H2(G,D) —> H2(H,D) is zero.
Proof. Let ? G H2(G,D) and 04D->M4(?->lbea central
extension corresponding to ?. To res (?) corresponds a central extension
A1.14) 0->D4]V4i?4l.
Since H C G', N C tj-^G') = M'?>. Also H C C(G); the centre of G
implies that AT C Q2(M)D. Q2(M) the second term in the upper central
series of M. Therefore 7V''c [M'D, B(M)D] = [M1 ,C,2(M)\ = 1. This
shows that AT is Abelian. Thus A1.14) is an exact sequence of Abelian
groups with D divisible Abelian. Therefore the sequence A1.14) splits and
res (?) = 0. Hence res : H2(G,D) -> H2(H,D) is the zero map. Q
A much better result is available.
Theorem 11.8.17 Let D be as above. If the subgroup H is central and
H IHHG' is cyclic, then res : H2(G, D) -> H2(H, D) is the zero map.
Proof. Choose an element x ? H such that H / H nG" is generated by
xHnG'. Then every element of H can be uniquely expressed as xra, where
a ? H fl G' and r is an arbitrary integer if H / H fl G' is infinite cyclic while
0 <r < to if H I HnG' is finite of order to (say).
Let z G H2 (G, D) and 0->D->M4G->lbea central extension
which corresponds to z. Then to res (z) corresponds a central extension
A1.15) 0->D->/V4ff"->l
where /V = n~1(H). Also image of z under
res : H2(G,D) -> H2(HnG',D)
is represented by the central extension
A1.16) 0->D->i4ffnG'->l
where L = TT~1(Hf]G'). Proposition 8.16 implies that the extension A1.16)
splits. Let ij) : H fl G' —> L be a homomorphism such that tt^ is identity
map onflfl G'. Observe that L C M'D is an Abelian group. Choose a
representative n G N of x G H arbitrarily and then choose a representative
4>{xra) of xra. G H by tf)(xra) = nrip(a). Since H is in the centre of G.
N CB(M)D. Then '
[n,i>(a)] G fa(M)D,M'D] = Co(M) = 1.
© 2003 by CRC Press LLC
Thus n commutes with if)(a) for every a G HC\G'. It follows that nrip(a) and
nstp{b) commute for all r, s or that <j>(h) and <j>(h') commute for all h, h' ?
H. Since elements of N are uniquely of the form d<j>(h). d ? D. h ? H and
D is a central subgroup of M (and. so. of N). N is an Abelian group. The
group D being divisible Abelian. the extension A1.15) splits which implies
that image of z under res : H2(G,D) —> H2(H,D) is zero, rj
Let us temporarily write Pn (G) for the nth term in the standard bar res-
resolution of Z regarded as a trivial (left) ZG-ma&n\e,. The natural projection
¦k : G —> GIH induces a ZG-homomorphism : Pn(G) —> Pn(G/H) for every
n which gives a chain map : P(G) —> P(G/iJ). Since H operates trivially
on Pn(G/H), every G-homomorphism: Pn(G/H) —> A takes values in AH.
Therefore, applying Homa(-,A) to the chain map : P(G) -»¦ P(G/H) we
get a commutative diagram
HomG/H(Pn(G/H),AH)
HomG(Pn-i(G),A) HomG(Pn(G),A)
HomG/H(Pn+1(G/H),AH) .-••
HomG(Pn+1(G),A) . ...
On taking homology this diagram induces for every n > 0 a homomorphism
inf : Hn(G/H,AH) -> Hn(G,A).
This homomorphism is called the inflation homomorphism.
Observe that image of inflation is contained in the kernel of restriction
:Hn(G,A) -> Hn(H,A).
Suppose that B is a right G-module on which H acts trivially or equiv-
alently R is a right G/iJ-module. Then
B ®G Pn(G/H) = B ®G/H Pn(G/H)
for every n. Tensor the chain map P(G) —> ~P(G/H) with B over G. We
get a commutative diagram
© 2003 by CRC Press LLC
Pn+1(G) ®G B - Pn(G) ®G B
Pn+1(G/H) ®G/H B Pn(G/H) ®G/H B
- Pn^(G/H) ®G/H B - ¦ • ¦
which on taking homology induces, for every n > 0. a homomorphism
Coinf : Hn(G,B) -> Hn(G/H,B).
This homomorphism is called the coinflation homomorphism.
Unfortunately, for n > 1, we donot have a homomorphism : Hn(H, A) -»¦
Hn+1(G/H,AH) although there exists a homomorphism : Hl(H,A)G ->
H2(G/H,AH). In order not to complicate matters, we define this homo-
homomorphism when iJ acts trivially on A.
Suppose that A is a G/iJ-module. Then
Hl(H,A)G = Hom(H,A)G ^ HomG(H,A) ^ Hom(H/[H,G],A).
Let {w(A)},\GG/ff be a right transversal of ff in G with w(l) = 1. Then
there exists a map W : G/H x G/iJ -»¦ ff such that
)(/); A, /i G G.
The associative law in G implies that
A1.17) {w{X)W{ii,v)w{\)-1)W{\,iiv) = W(X,n)W(Xfj,,v)
for all A. (i. v G G/iJ. Let / G Hom(H,A)G and apply it to the relation
A1.17). We get
XfW(n,v)+fW(X,nv) = fW(X,n) + fW(X»,v).
This shows that / W : G/H x G/H ->¦ A is a 2-cocycle. Define a map
* : Hom(H,A)G -> H2(G/H,A)
by ?(/) = [/W], / G Hom(H,A)G. It may be easily seen that t is a
homomorphism. This homomorphism is called the transgression homo-
homomorphism.
As an application, we state the following result without proof. (A proof
is omitted in order to avoid considering spectral sequences.)
© 2003 by CRC Press LLC
Theorem 11.8.18 If H is a normal subgroup of a finite group G with index
[G : H] = m (say) coprime to the order k of H, then for each G-module A
and each n > 0, there is a split exact sequence
0 -> Hn(G/H, AH) lH Hn(G, A) r4 Hn(H, A)G -> 0
and
Hn(G, A) =s Hn(G/H, AH) © Hn(H, A)G.
11.9 Some Exact Sequences
Let G be a group. H be a normal subgroup of G and A be a left G/H-
module. Homology and cohomology of G are related to respectively ho-
mology and cohomology of the subgroup H and the quotient group G/H
through a spectral sequence. To keep our treatment elementary and as
simple as possible we donot treat spectral sequences in this text. However,
the low degree terms of the spectral sequence yield 5-term exact sequences.
In this section we study these sequences, certain extensions and some ap-
applications of these sequences. Unless explicitly stated otherwise. G. H and
A are as above. Also {w(X)}xeG/H with w(l) = 1 denotes a set of left
coset representatives of the elements of G/H in G so that every element
of G is uniquely represented in the form hw(X), h G H, A G G/H and
W : G/H x G/H ->¦ H is the associated function:
w{X)w(fj,)=W(X,fj,)w{Xfj,): X,(ieG/H.
The map W satisfies the relation A1.17) i.e.
(w(X)W((j,, v)w(X)~ )W(A,/iv) = W(X,ju)PF(A/i, v)
for all A. fj,. v G G/H.
Theorem 11.9.1 There is a natural exact sequence
A1.18) 0 -> Hl(G/H,A)l^i Hl(G,A)r-? HomG(H,A)
4 h
Proof, (a) Let / : G/H -> A be a 1-cocycle. / : G -> A be denned by
f(x) = f(xH). x G G and a G A such that f(x) = xa - a. As A is acted
upon by H trivially, xa - a = (xH)a - a or f(xH) = (xH)a - a which
shows that / is a 1-coboundary. Thus inf : H\G/H,A) -> Hl(G,A) is a
monomorphism.
It is clear that for every n > L Hn(G/H, A) %H Hn(G, A) 4s Hn(H, A)
is a zero sequence. Let / : G -»¦ A be a 1-cocycle. Suppose that / | H = 0.
For x eG, he H, f(xh) = xf(h) + f(x) = f(x). Therefore / induces a
© 2003 by CRC Press LLC
well defined map / : G/H -> A given by f(xH) = f[x). x G G. The map
/ is a 1-cocycle and image of the cohomology class [/] under inflation is the
cohomology class [/]. This completes the proof that the sequence
0 -> Hl (G/H, A)H H\G, A) r4s HomG (H, A)
is exact.
(b) Let / : G -»¦ A be a 1-cocycle and define g : G/H -»¦ A by g(X) =
fw(X), A G G/H. For A; n G G/H,
Sg(X,/i) = Xg(fi) - g(Xfi) + g(X)
= Xfw(fi) - fw(Xfi) + fw(X)
= Xfw(fi) — /(PF(A,ju)~1ui(A)ui(ju)) + fw(X)
= Xfw(fi) — W"(A, yu) f'(w(X)wi(fJ,)) — fW(A, yu) + fw(X)
= Xfw(fi) - f(w(X)w(fj)) + fw(X) + fW(X, n)
(as f restricted to H is a homomorphism)
= 6f(w(X)Mn))+fW(X,fi)
This proves that (tores)([f\) = 0 or that image of res : HY(G,A) -»¦
Homa(H, A) is contained in the kernel of the transgression map t : Homa(H,
A)-> H2(G/H,A).
Next, let. / G HomG(H,A) such that <([/]) = [fW] = 0. Then there exists
a map g : G/H —> A such that fW = Sg. Define a map / : G —> A by
J(hw(X)) = g(X) + f(h), A G G/H, h?H.
For h, k?H, X, fi ? G/H,
6j(hw(X),kw(ii))
= hw(X)J(kw(n)) -J(hw(X)kw(n)) +J(hw(X))
= w(X)J(kw(fi))-J(hw(X)kw(X)-1W(X,fi)w(Xli))+J(hw(X))
= w(X)g(fi) - g(Xfi) + g(X) + w(X)f(k) - f(hw(X)kw(X)~1)
= Sg(X,fi) + fiw^kwiX)-1) - f(h) - ftwWkwiX)-1) - fW(X,fi)
+f(h)
= Sg(X,fi)-fW(X,fi)=O.
Therefore/ is a 1-cocycle. For h G H, f(h) = f(h) which shows that
/| H = f. Therefore, res ([/]) = /. This proves that kernel of t :
Homa(H, A) -»¦ H2(G/H, A) is contained in the image of res : H1 (G, A) -»¦
Homa(H,A). Hence the sequence
H\G,A) r4s HomG(H,A) 4 H2(G/H,A)
© 2003 by CRC Press LLC
is exact.
(c) Let / G Ho7tig(H,A). Then inf ot([f]) is represented by a 2-cocycle
/ : G x G -»¦ A given by
J(hw(X),kw(n)) = fW(X,n), X: 11 G G/H, h, k G H.
Define g : G -> A by g(hw{X)) = -f(h): h ? H: A G G/#. For A; /x G
G/#. h,k?H,
8g(hw{X), kw(fj,))
= hw(X)g(kw(fi)) — g(hw(X)kw(fj,)) + g(hw(X))
= -w(X)f(k) - g(hw(X)kw(X)-lW(X,ii)w(Xii)) - f(h)
= -w(X)f(k) + f(h) + f(w(X)kw(X)-1) + fW(X,n) - f(h)
= fW(X,ix)
= J(hw(X),kw(fi)).
Thus / = 6g is a coboundary and. therefore, inf ot([f]) = 0.
Next, suppose that / : G/H x G/H ->¦ A is a cocycle such that / :
G xG ^ A defined byJ(hw(X),kw((j,)) = f(X,(i), X,n? G/H, h,k?H,
is a coboundary. Let f = Sg for a map g : G —> A. Define ~g : H —>¦ A
by g(h) = g{h)., h G H. Observe that 6g(h, k) = 0 for all h, k ? H which
shows that g | H and. therefore. ~g is a homomorphism. Again . for AgG/H.
hGH;
Therefore
0 = 8g(w(X),h)
= Xg(h) - g(w(X)h) + g(w(X))
= Xg(h) - 5(W(A)/lW(A)W(A)) + g(w(X))
= Xg(h) - \w(X)hw(X)-lg(w(X)) + g(w(X)hw(Xy1)} + g(w(X))
= Xg(h) - gw(X) - giw^hwiX)-1) + gw(X)
which implies that g(w(X)hw(X)~1) = Xg(h). This shows that ~g : H —»¦ A
is a G-homomorphism. Define a map 7j : G/H —> A by <?(A) = p(i/;(A)), A G
G/H. For A; /x G
= A(?(w(/i)) - g(w(Xfi)) + g(w(X))
= Xg(w(fi)) - g{W{X, ^)-lw{X)w^)) + g(w(X))
= Xg(w(fi)) - g{W{X, /x)) - g(w(X)w(fi)) + g(w(X))
= Sg(w{X),w{fj,))+gW{X,fj,)
© 2003 by CRC Press LLC
showing that [/] = t(—g). i.e.. Ker(inf) C Im{t). This proves that the
sequence
HomG(H,A) -4 H2(G/H,A) % H2(G,A)
is exact.
Combining all the bits together, we have proved that the sequence
A1.18) is exact. Since the homomorphisms inf and res are functorial and
we can prove easily the functoriality of the transgression homomorphism.
the sequence A1.18) is natural exact, rj
We can now prove the dual of Hopf formula for the second cohomology
group. So far we have taken B. to be a ring with identity. Tn this section
and,may be. the rest of this chapter, we do not insist on R being a ring. For
a group K. let. K' denote the derived group of K and when H is a normal
subgroup of K. let [H, K] denote the (normal) subgroup of K generated by
all elements of the form [h,k] = h~lk~1hk, h G H. k G K. Let G be a
group and 1—> B. —> F —> G —>¦ 1 be a free presentation of G i.e. F is a
free group and B is a normal subgroup of F with G = F/R. Let D be a
divisible Abelian group regarded as a trivial G-module.
Theorem 11.9.2 The second cohomology group H2(G,D) is isomorphic
to Hom(RnF'/[R,F],D).
Proof. Since D is a trivial G-module. Hl(G,D) = Hom(G,D) and the
exact sequence A1.18) associated with the exact sequence 1—> B —> F —>
G —> 1 takes the form
0 -> Hom{G, D) lH Hom{F, D) r4s HomF{R, D)
2(G,D)^ H2(F,D).
t
Since F is a free group, H2{F, D) = 0. Also Hom{F, D) ^ Hom(F/F', D),
HomF{R, D) ^ Hom(R/[R, F],D) and the homomorphism Hom(F/F', D)
—> Hom(R/[R, F], D) is the one induced by the inclusion map. Now D be-
being a divisible Abelian group (so an injective Z-module). Hom(—,D) is an
exact functor. Applying this functor to the exact sequence.
l->fifl F'/[R, F] -> R/[R, F] -> R/R n F' -> 1
we get an exact sequence
0 -> Hom(R/Rr\F',D)^ Hom(R/[R,F],D)
-> Ham(RnF'/[R,F],D)->0
Consider the commutative diagram
© 2003 by CRC Press LLC
Hom(F/F', D) r-tsHom{R/[R, F],D)-L H2{G, D)
0~Hom(R/R n F', D)—¦ Hom(R/[R, F], ?>)— Hom(R n F'/[R, F],
in which the rows are exact and the vertical homomorphism on the left is
induced by the monomorphism R/R nF'-> F/F'. There is then induced
a homomorphism 0 : H2(G,D) -> Hom(R n F'/[R,F], D) which makes
the completed diagram commutative. An easy diagram chasing then shows
that 0 is an ismorphism.rj
We next consider two extensions of the 5-term sequence A1.18).
Let H2(G, A)*H denote the kernel of the restriction homomorphism res :
H2(G,A) -> H2(H,A).
Proposition 11.9.3 //? G H2(G,A)*H, there exists a 2-cocycle f : G x
G —> A which represents ? and satisfies
A1.19) f(hw(X),kw(n)) = f(w(\),w(n)) + f(w(X)),k)
A1.20) f(x,hk) = f(x,h) + f(x,k)
for all X,fi? G/H, xGG., h..k?H.
Proof. Let 0 —> A —> M A- G —> 1 be an extension corresponding to
?. Let N = tt~1(H). Then the extension 0->A->iV4iJ->l which
corresponds to res (?) splits. Let <f> : H -»¦ N be a splitting homomorphism.
For A G G/H choose a representative <j)(w(X)) G M arbritrarily and set
<j)(hw(X)) = <t>{h)<t>{w{X))., h?H: A G G/H
Let / : G x G -»¦ A be the 2-cocycle corresponding to this choice of repre-
representatives in M of the elements of G. Then
(i) f(h, k) = 0 (m)/(/i, w(A)) = 0 for all h..k?H:X? G/H.
For h: k?H: A G G/ff
/(ft, MA)) = -/(*, «^(A)) + f(hk, w(X)) + f(h, k) = 0.
Thus for all h G H, x G G. f(h, x) = 0. Now for h, k G H, X. n G
(a) f(hw(X),kw(n)) = f(w{X),kw(fj))+f(h,w(X)kw(fj))-f(h,w(X))
© 2003 by CRC Press LLC
= f(w(X),kw([i))
= -w(\)f(k, w{ii)) + f(w(X)k, w(n)) + f(w(X),k)
= f(w(X)kw(X)-1w(X),w(fx)) + f(w(X), k)
= f(w(X),w(fj,)) + /(u)(A)fcu)(A)~1,u)(A)ui(ju))
-f(w(X)kw(X)-\w(X)) +f(w(X),k)
= f(w(X),w(ij))+f(w(X),k)
which proves A1.19).
(b) f(x,hk) = -xf(h,k) + f(xh,k) + f(x,h)
= f(xhx~1xJk) + f(x,h)
= f{x, k) + f(xhx~l, xk) — f(xhx~l, x) + f(x, h)
= f(x,k) + f(x,h) = f(x,h) + f(x,k)
which proves A1.20). q
Remark 11.9.4 // N in the above proof is contained in the center of M,
then necersarily H is contained in the center of G and for h ? H. X ? G/H,
= f(w(X), h
= f(w(X),h)cf>(hw(X)).
Therefore f(w(X), h) = 0 for all h G H, X G G/H.
Let /:GxG->4bea 2-cocycle satisfying properties A1.19) and A1.20).
For A G G/H, define /(A) : H -> A by
7(A)(/j) = f(w(X),w(X)-lhw(X)), h?H.
It follows from A1.20) that /(A) is a homomorphism. We thus have a map
/ : G/H —> Hom(H, A). Since A is an Abelian group, every homomorphism
: H —»¦ A gives rise to unique homomorphism : H/H' —> A and coversely.
Also because of the property A1.20) satisfied by /. we can continue to work
with H rather than H/H'.
Lemma 11.9.5 The map f is a 1-cocycle. Moreover, if g is another 2-
cocycle satisfying A1.19) ,A1.20) and cohomologous to f, then f, ~g are
cohomologous.
Proof. Let A, /j. G G/H. For any he H,
(Xj(fi)-J(Xfi)+J(X))(h)
+J(X)(h)
© 2003 by CRC Press LLC
+/(w(A),w(A)/iw(A)) (because of A1.20))
= \f{w{ij),w{\iJL)-lhw{\iJL)) - f(W(X,i^)w(Xfi),w
+f(w(X)Jw(X)~1hw(X)) (because of A1.19))
l hw(Xfi))
+f(w(X),w(X)-1hw(X))
= -f(w(X),w(fx)w(Xfi)-1hw(Xfx))+f(w(X),w(fi))
+f(w(X),w(X)-1hw(X))
= -/(w(A),w(Ju)w(AJu)/iw(AJu)w(Ju)w(yu)) + f(w(X),w(fi))
+f(w(X),w(X)-lhw(X))
A1.19))
+f(w(X),w(X)-1hw(X))
= -f(w(X)Jw(X)-1hw(X))+f(w(X),w(X)-1hw(X))
= 0.
This proves that A/(/z) - /(A/x) + /(A) = 0 and hence / is a 1-cocycle.
Since g is cohomologous to /. there exists a map tp : G —»¦ A such that
f — g = Sip, where S is the coboundary operator. From A1.19) it follows
that ip \fj is a homomorphism. For A ? G/H, h ? H,
= f(w(X),w(X)-lhw(X)) - g(w(X),w(X)-lhw(X))
= (f-g)(w(X),w(X)-1hw(X))
= SiP(w(X),w(X)-1hw(X))
= w(A)V'(w(A)-1/iw(A)) - iP{hw{X)) + ip(w(X))
A1.21) = (XiP)(h)-iP(hw(X))+iP(w(X)).
Again, since /. g satisfty A1.19).
0 =(/-(?)(h, w(X)) = SiP(h, w(X)) = iP(w(X)) - iP(hw(X)) + iP(h).
Using this in A1.21). we get
G - g)W(h) = (Xip)(h) - ip(h) = (Xip - ip)(h) = (Sip)(X)(h).
This gives G - <?)(A) = Sip(\) for every A G G/H. Thus J-g = 5ip which
proves that /. g are cohomologous. rj
© 2003 by CRC Press LLC
Let ? G H2(G, A)*H and / : G x G -> A be a 2-cocycle satisfying A1.19),
A1.20) which represents ?. It follows from Lemma 9.5 that/ gives a well
defined element [/] of H1(G/H,Hom(H,A)) which is independent of the
choice of f. We define
9 : H2(G,A)*H -> Hl{G/H,Hom{H,A))
by
A1.22) 0@ = [/].
It is fairly easy to check that. 0 is a homomorphism.
Proposition 11.9.6 Kernel of the homomorphism 0 is contained in the
image ofinf : H2(G/H,A) -> H2(G, A).
Proof. Let ? G iJ2(G,A)^ such that 0@ = 0. Let / be a 2-cocycle
representative of ? satisfying A1.19) and A1.20). Then there exists a ho-
homomorphism tp : H —> A such that
/(A) = XiP-tp., A G G/H.
Define a map x : G —>¦ A by
x(/wu(A)) =V(/i): h?H., X&G/H.
For h: k,GH: X: iiG G/H.,
(f-SX)(hw(X),kw(fi)) = f(w(X),w(jjL))+f(w(X),k)-XX(kw(ji))
+x(hw(X)kw(n)) - x(hw(X))
= f(w(X),w(fi))+f(w(X),k)-X^(k)
A1.23) = f(w(X),w(»))+f(wW,k)-Xil>(k)
+iP(w(X)kw(X)-1)
Now
f(w(X),k) = J
= Xtp(k) - iP(w(X)kw(X)-1)
or
f(w(X), k) - Xi/>(k) + tP(w(X)kw(X)-1) = 0
Using this in A1.23) we get
(/ - SX)(hw(X), kw(fi)) = f(w(X),w(n))
© 2003 by CRC Press LLC
Set g = f — 8x- Then g is also a 2-cocycle representative of ? and
g(hw(X), kw(fi)) = f(w(\),w(»)) + W{\ »)¦
The right hand side being independent of h. k. we also have
Therefore
A1.24)g(hw(X),kw(fj,)) = g(w(X),w(fj,)) for all h.. k G H, X.,
Define g : G/H x G/H -> A by
For A, fi, v G G/if,
Xg(p, v) - g(X ii, v) + g(X, fiv) - g(X, fi)
= Xg(w(fi),w(v)) - g(w(Xfi),w(v))
+g(w(X),w(fj,v)) - g(w(X),w(fj,))
= Xg(w(fi),w(v)) - g(W(X, fj,)w(Xfi),w(v))
+g(w(X), W(n, v)w(fiv)) - g(w(X)w(ii)) (by A1.24))
= Xg(w(fj,),w(v)) - g(w(X)w(fj,),w(v))
+g(w(X),w(fj,)w(v)) - g(w(X),w([i)
= 0. as g is a cocycle.
Thus g is a 2-cocycle . Let r/ be the element of H2(G/H,A) given by g.
The definition of inflation shows (in view of the relationship between g and
g) that inf (r/) = [g] = [/] = ?. This completes the proof, q
Let rj G H2(G/H,A) and g : G/H x G/H ->ibea 2-cocycle which
gives r/. Let inf (r/) = ?. Then ? is given by a 2-cocycle f : G x G -> A.
where
f(hw(X),kw(fi)) = g(X,li), h, k G H.. X., /i G G/H.
Evidently / satisfies the properties A1.19) and A1.20) and for h G H, X G
G/H.
This proves that 0(?) = [/] = 0. Hence image of inf : H2(G/H,A)
H2(G, A) is contained in the kernel of
6>: H2(G, A)*H -+ Hl{G/H, Hom(H, A)).
Combining this with Proposition 9.6. we have
© 2003 by CRC Press LLC
Theorem 11.9.7 The sequence
H'2(G/H,A) H H2{G,A)*H 4 Hl{GIH,Hom{H,A))
is exact.
Tf 77 is a central subgroup of G and A is a trivial G-module. then
Hom(H, A) becomes (under the diagonal action) a trivial G-module and
H1 (G/H, Hom(H, A)) = Hom(G/H, Hom(H, A)) = Hom(G/H ®H,A).
Corollary 11.9.8 // H is a central subgroup of G and A is a trivial G-
module, then the sequence.
H2(G/H,A) H H2{G,A)*H -4 Hom(G/H (8 H,A)
is exact.
From now on we suppose that. G is a nilpotent group of class n. H = Gn -
the nth term in the lower central series of G and A is a trivial G-module.
Let Cm(G) denote the mth term in the upper central series of G.
Theorem 11.9.9 There exists a homomorphism
p:H2(G,A)*Gn -^Hom(G/Cn-i(G)<S>Gn,A)
such that the sequence
H2(G/Gn, A) H H2(G, A)*G^ 4 Hom(G/Cn-i (G) ® Gn, A)
is exact.
Proof. Let ? G H2(G,A)*Gri and 0->A->M4G->lbea central
extension corresponding to ?. Let <f> : G —> M be the map and / : G x G —>
A be the 2-cocycle as in Proposition 9.3. For h ? Gn. 4>{h) ? MnA and for
zGCn-itG), ^(a:) GCn(M). Also
[MnA,U(M)] = [Mn,(n(M)} C Co(M) = A).
Hence
<t>{h)<t>{x) = <t>{x)<t>{h) for all h G Gn, x G Cn-i(G).
This implies
f(x,h) = f(h,x) = 0 for all h G Gn,x G Cn-i(G).
The homomorphism / : G/Gn —> Hom(Gn,A) (cf. Lemma 9.5) thus van-
vanishes on Cn-i(G)/Gn. Hence it induces a homomorphism / : G/?n-i(G) -»¦
© 2003 by CRC Press LLC
Hom(Gn,A). Since / is independent of the choice of the 2-cocycle /. so is
/ and we get a homomorphism
<P:H2(G,A)*Gri -> Hom(G/Cn-i(G),Hom(Gn,A))
= Hom(G/Cn-i(G)®Gn,A)
making the diagram
inf
J
inf 0
H2(G/Gn,A) J—» H2(G,A)*Gn * Hom(G/Gn®Gn,A)
Hom(G/Cn-i(G)(i>Gn,A)
A1.25) 0
commutative.
Here the homomorphism j being induced by the natural projection
G/Gn —> G/?n_i(G) and the identity map 1 : Gn —> Gn is a monomor-
phism. The row in the diagram A1.25) being exact and j being a monomor-
phism. the sequence
H2(G/Gn, A) H H2(G, A)Gn % Ham(G/Cn-i (G) ® Gn, A)
is exact, q
Let G and A be as in the above theorem. Then H1 (G, A) = Hom(G, A)
and res : Hom(G,A) -»¦ Hom(Gn,A) is the zero homomorphism. Using
this and combining the sequence of the above theorem with the 5-term
sequence A1.18). we get
Theorem 11.9.10 Let G be a nilpotent group of class n and A be a trivial
G-module. Then the sequence
0 -> Hom(Gn, A) 4 H2(G/Gn, A) lH H2(G, A)*Gn
-> Hom(G/{n-1(G)®Gn,A)
is exact.
When A = T. the additive group of rationals mod 1 regarded as a trivial
G-module; res : H2(G,T) -> H2(Gn,T) is zero (Proposition 8.16) and in
the above sequence (with A replaced by T) H2(G, A)Gn becomes H2(G, A).
© 2003 by CRC Press LLC
We again return to the case when G is any group. H is a central sub-
subgroup of G and A is a trivial G-module. We define a homormorphism
p : Hom(G/G'H ®H,A)^ H'S(G/H, A) and extend the exact sequence of
Corollary 9.8 by one term.
Let V G Hom(G/G'H <8 H,A). Define / : G/H x G/H x G/H -> A
by
f(\,H,v) =ip(w(\)G'H<Z>W(n,v))., X,h,ugG/H.
For A. fi, v, rj G G/H,
f{li.. v, n) - /(A (i, v, n) + f(X, fn>..r})- /(A: ^; 2^77) + /(A: (i, v)
®W(v., r]))-ip(w(\fj,)G'H®W(v.. 77))
(8 W(/iu, v)) ~ i>(w(X)G'H (8 W(^; v rj))
+ip(w(X)G'H®W(n,v))
= i/}{w(ij)G'H (8 W(v, v)) ~ i>(w(X)G'H (8 W(v, v))
-ip(w([i)G'H ® W(v,rj)) + tp(w(X)G'H ® W(nv: r/))
-ij}{w{X)G'H ® W(fi, v rj)) + ip(w(X)G'H ® W(fi, v))
= i(}(w(X)G'H ®(-W(v,r]) + W(/j,v, rj) -W(/j,, vrj) + W(/j,, v))
= 0. as W is a 2 — cocycle.
Thus / is a 3-cocycle. We define p by
It is fairly easy to check that p is a homomorphism.
Theorem 11.9.11 The sequence
H'2(G, A)*H A Hom(G/G'H ®H,A)A H'S(G/H, A)
is exact.
Proof. Let ip e Hom(G/G'H ® H,A). Then p(V>) = [9], where
g : G/H x G/H x G/H -> A is defined by
p(A: ^; u) = ij}{w{X)G'H ® W(ii.. v)).. I/i;u? G/H.
Suppose that, p(^) = 0. Then there exists a map 7 : G/H x G/H
such that, g = Sj i.e.
i, v) + 7(A. jiv) - 7(A. jj), X.fi.uG G/H.
Define a map / : G x G -»¦ A by
f(hw(X),kw(fj,)) = ij}{w{X)G'H ®k)- 7(A: ^): h., k ? H: X., n ? G/H.
© 2003 by CRC Press LLC
For h, k, I G H, X, n,u? G/H,
(a) f(kw(p), lw{y)) - f((hkW(X., n))
+f(hw(X),klW(n, v)w{iiv)) - f(hw(X),kw(fi))
= tp(w(fi)G'H ®l)- t/j(w(X)w(fj,)G'H ®l)+ ip(w(X)G'H ® klW(n, v))
-ip(w(X)G'H ® k) - j(fi. v) + 7(A,u. v) - 7(A. fj,u)+ j(X. fj)
= iP(w(X)G'H (g, W([i.. i/)) - g(X: ^ v)
= g{X, fi. v) - g(X, fi. v) = 0.
Therefore / is a 2-cocycle.
(b) f(hw(X),kw(fi)) - f(w(X),w(p)) - f(w(X), k)
= ip(w(X)G'H «) k) - 7(A: fi) + j(X, fi) - i/>(w(X)G'H ® fc) = 0.
This shows that / satifies A1.19). That / satisfies A1.20) is also easy to
prove. Let 6([f] = X- By definition of 9.
x(w(X)G'H ®k) = f(w(X), k) = i/>(w(X)G'H ® k)
which proves that \ = ip i-e- ip = ^([/])- Hence Ker p C Im 9.
Next, let ^ ? H2(G,A)*H and / be a 2-cocycle which represents ? and
satisfies A1.19) and A1.20). Then 0(?) = ip where t/}(w(X)G'H & k) =
f(w(X),k) for A G G/H, k G H. If p(tp) = [g], the cocycle g is given by
A1.26) g{X, II, v) = i/j(w(X) <E> W(n, v)), X, fi, v G G/H
= f(w(X),W(fi..v)).
Define x ¦ G/H x G/H -> A by
X(A; /x) = -/(w(A),«;(/*)), A, M G G/H.
Then, for X, n, v ? G/H,
-,v) -x{XH; v) +x{\ l*v) -x(A. fj)
= -f(w(fi),w(v)) +f(w(Xfj,),w(v)) - f(w(X),w(fiv))
= -f(w(p), w(v)) + f{W{\,n)w{\n), w{v))
-f(w(X), W(n, v)w{liv)) + f(w(X),W(fi, v)) + f(w(X),w(ri)
(because of A1.19))
= -f(w{p),w(v)) + f(w(X)w(n),w(v)) - f(w(X),w(n)w(v))
+f(w(X),w(ii)) + f(w{X),W(fi,v))
= /(w(A), W(fi, v)) (as f is a cocycle)
= g(X,n,v) (by A1.26))
This proves that, g is a coboundary and pty) = 0. Hence Im 9 C Ker p.
© 2003 by CRC Press LLC
Theorem 11.9.12 Let G be a milpotent group of class n and A be a trivial
G-module. Then there is defined a homomorphism
a : Hom(G/Cn-i(G) ®Gn,A) -> H3(G/Gn,A)
such that the sequence
0 -> Hom(Gn, A) -4 H2(G/Gn, A) lH H2(G, A)*Gn
% Hom(G/Cn_i (G) ®Gn,A)^H3 (G/Gn, A)
is exact.
Proof. Consider the commutative diagram (cf diagram A1.25))
H2(G,A)*Gn JL^ Hom(G/G'®Gn,A) —^ H3(G/Gn,A)
Hom(G/Cn-i(G)®Gn,A)
0
in which the row is exact ( Theorem 9.11) and j is a monomorphism. Take
a = pj. An easy diagram chasing shows that Imtp = Kera so that the
sequence
H2(G, A)*Gn % Hom(G/Cn-i(G) (8 Gn,A) ^ H3(G/Gn,A)
is exact. Combining this sequence with the exact sequence of Theorem
9.10. we get the desired exact sequence, q
We close this section with a short, exact sequence which is quite useful
in the computation of the Schur multiplicator of a group.
Let G be a group with a free presentation l—>R—$-F^>G—>l. where
F is a non-cyclic free group and R. a normal subgroup of G. Let T be the
additive group of rationals mod 1 regarded as a trivial G-module.
Proposition 11.9.13 There, is a split exact sequence
0 -> Hom(R/Rn F',T) 4 Hom(R/[R,F],T) A H2(G,T) -> 0.
Here F1 denotes the derived group of F.
© 2003 by CRC Press LLC
Proof. Since R is a normal subgroup of F. [R, F] is a normal subgroup
of R. Therefore we have an exact sequence
A1.27) 1 -> R n F'/[F, R] -> R/[F, R] -> i?/i? n F' -> 1
where the first map is the inclusion homomorphism and the second map is
the natural projection. Observe that. R/R f] F' = RF'/F' which being a
subgroup of F/F' is free Abelian. Therefore the exact sequence A1.27) of
Abelian groups splits. Applying the functor Hom( — ,T) to the sequence
A1.27) we get a split exact sequence
0 -> Hom(R/RnF',T)^ Hom(R/[F,R],T) ^
Hom(RnF'/[F,R],T) -> 0.
We have seen earlier that Horn (RC\F'/[F, R],T) ^ H2(G, T) and the result,
follows, rj
11.9.14 Exercises
1. If H is a normal subgroup of G and A is a G/H-module. prove that.
Hom(H, A) is a G/H-module under the diagonal action.
2. Prove that the map 9 : H2(G,A)*H -> Hl(GIH,Hom(H,A)) defined by
A1.22) is a homomorphism.
3. If H is a central subgroup of G and A is a trivial G-module. prove that.
Hom(G/HG', Hom(H, A)) ^ Hom(G/HG' ®H,A).
4. If _H" is a central subgroup of G and A is a trivial G-Module. prove that
(a) Hom(H, A) is a trivial G-module (under the diagonal action) and
(b) Hl{GIH,Hom{H,A)) ^ Hom(G/G'H ® H,A).
5. Prove that the transgression homomorphism t : HomoiH, A) -»¦ H2(G/H,
A) is natural.
6. Are the homomorphisms
and
it : Hom{GlQn-l{G)®Gn,A) -> H
functorial ?
7. If G is a non-Abelian group of order p3, p a prime, prove that G' =
is a cyclic group of order p and G/G' = Zp® Zp.
8. If G is a finite Abelian group and T is the additive group of rationals
mod 1. prove that. Hom(G,T) ^ G.
9. Let H be a normal subgroup of a group G and A be a G-module.
Define transgression homomorphism t : ^{H, A)G —> H2(G/H,AH) and
prove that the sequence
H\G,A) r4s Hl(H,Af A H2(G/H,AH) H H2(G,A)
is exact.
© 2003 by CRC Press LLC
Chapter 12
Some Applications
In this chapter we give some applications of homological methods to purely
group theoretic problems.
12.1 An Exact Sequence
Tn this section we define induced and coinduced modules, prove Shapiro's
lemma and obtain an exact sequence for cohomology which is needed in the
next section.
Let G be a group. H be a subgroup of G and A a left ZiJ-module.
Consider the Abelian groups ZG®h A and HoniH(ZG,A). For x. y ? G.
a ? A and / ? HomniZG, A) we define
y(x <8> a) = (yx) C3 a.
With this action ZG ®h A and HomniZG, A) become left ZG-modules.
Definition 12.1.1 A ZG-modu\e A is called an 77-induced module if
there exists a ZH-module X such that. A = ZG ®h X and is called
a _H"-coinduced module if there exists a ZiJ-module Y such that. A =
HomH(ZG,Y).
Let A be a left ZG-module. There is a well defined homomorphism
¦k : ZG <3h A —»¦ A. which on the generators of ZG <Sih A is given by
ir(x <8> a) = xa. x ? G. a ? A.
Define a map i : A —> ZG <S>h A by i(a) = 1 ® a. a ? A. The map i is
trivially an H-homomorphism and ir i = 1a- Observe that although n is a
293
© 2003 by CRC Press LLC
ZG-homomorphism. i is in general not a G-homomorphism. Therefore the
sequence
0 -> K -> ZG ®H A 4 A -> 0:
where /<f = ATer ?r splits as a sequence of ZJJ-modules.
For a € A. define fa : ZG ->¦ A by /a(z) = a;a, ? G ZG. The map fa
is a ZG-homomorphism. in particular an iJ-homomorphism. The map a :
A —> Hottih(ZG,A) defined by a(a) = fa, a ? A. is a Z-homomorphism.
For x, y G G. aG A
(yfa)(x) = fa(xy) = (xy)a = x(ya) = fya(x).
Therefore a(ya) = fya = yfa = ya(a) and a is a G-homomorphism. Define
a map /3 : HomH{ZG,A) -> A by /?(/) = /A): / G HomH{ZG,A). For
any /?. G -ff. / G HomH{ZG, A).
P(hf) = ihf){\) = f(lh) = f(h) = hf(l) = hC(f)
and /3 is an _H"-homomorphism but not. in general, a G-homomorphism. For
any a ? A. /3a(a) = j3(fa) = fa(l) = la = a. Therefore /3a = 1a and the
sequence
0->A4 HamH(ZG, A) ->/",-> 0:
where L = Coker a. splits as a sequence of ZJJ-modules.
Definition 12.1.2 A left ZG-module A is called relatively H-projective
(or (G,H)-projective) if A is a direct factor of ZG®h A as a ZG-module
and A is called relatively H-injective (or (G,H)- injective) if A is direct
factor of HomniZG^A) as a ZG-module.
If the subgroup H of G is 1. the .ff-induced and .ff-coinduced modules
are the usual induced and coinduced modules respectely. Also, then the
(G, .ff )-projective and (G, .ff )-injective modules are the usual weakly pro-
jective (or relatively projective or G-special) and weakly injective
(or relatively injective) modules respectively.
Proposition 12.1.3 (Shapiro's lemma) . // A is a left ZH-module,
then,
Hn(G, HomH(ZG, A)) =s Hn(H, A) and Hn(G, ZG ®H A) =s Hn(H, A)
for all n>0.
Proof. Let P be a projective resolution of Z as a right ZG-module.
Since ZG is a free ZH-module (with a transversal of H in G as a basis). P
is also a projective resolution of Z over ZH. There is then an isomorphism
of complexes
h A) ^P®HA.
© 2003 by CRC Press LLC
Therefore, the homologies of the two complexes are isomorphic i.e.
Tor^G{Z,ZG®H A) - Tor*H(Z,A)
or
Hn{G,ZG®HA)s*Hn(H,A).
Next, take a ZG-projective resolution P of Z as a left ZG-module. Then
P is also a ZJJ-projective resolution of Z as a left ZiJ-module. Theorem
3.4.5 then induces an isomorphism of complexes
HamG(P,HomH(ZG,A)) ^ HomH(P,A).
Therefore the homologies of the two complexes are isomorphic and we have
Hn(G,HomH(ZG,A)) =s Hn(H,A)
for all n > 0. rj
Corollary 12.1.4 // B and C are respectively induced and coinduced left
ZG-modules, then
Hn(G,C) = 0 and Hn(G,B) = 0 for all n > 0.
Corollary 12.1.5 If A and C are respectively relatively protective and rel-
relatively injective left ZG-modules, then
Hn(G,C)=0 and Hn(G,A)=0 foralln>0.
Proposition 12.1.6 // G is a group, H is a subgroup of finite index in G
and, A is a left ZH-module, then ZG ®h A and Hottih(ZG, A) are isomor-
isomorphic as ZG-modules.
Proof. Let S = {s\, ¦ ¦ ¦, sm} be a left transversal of H in G with 1 G S.
Then ZG is a free right ZiJ-module with S as a basis. Also S = {s~1 \s ?
S} is a right transversal of H in G and ZG is a free left ZH-module with
S as a basis. Define a map
)^ ZG®HA
by
0{f) = Y.s®f^1)-- f?HomH(ZG,A).
ses
Since / is an iJ-homomorphism. the element Y^ s ® f(s~1) is independent
of the choice of the transversal S of H in G. The map 9 is clearly a
homomorphism of Abelian groups.
Let x GG. Then
xes ses
© 2003 by CRC Press LLC
The subset {x 1s\s G S} of G is a left transversal of H in G. Therefore
Es0~ls) ® /(s^) = Ess ® /0) and 0(z/) = z0(/). Hence 6> is a
ZG-homomorphism.
Given an element (Efcis^i>a) e -^ x A where Aj G Z_ff\ define
/ : ZG -> A by
The map / is clearly a Z_ff-homomorphism. We then define a map
6 : ZGxA^ HomH(ZG, A)
by
6(^2 Si\i,a)(^2 fijSj1) = ^/ijAja. /z, G
The map 0 is bilinear and so induces a homomorphism
6 : ZG®H A^ HomH(ZG,A).
Let / G HomH(ZG,A). For fij G ZH.,
Therefore 68 is the identity map on HoniH{ZG,A).
On the other hand, for at G A.
(E
* .7
showing that 86 is the identity map of ZG®h A. Hence 8 is an isomorphism
of ZG-modnles. rj
As a particular case of the above result it follows that the concepts of
induced and coinduced module are identical for a finite group G. We then
have
Corollary 12.1.7 // G is a finite group and A is any Abelian group, then
Hn(G, ZG®A) = tt for all n > 0.
We shall need the following result in the next section.
© 2003 by CRC Press LLC
Theorem 12.1.8 Let H be a normal, subqroup of a group G, A a G-module
and q be a positive integer such that Hl(H, A) = 0 for 1 < i < q — 1. Then
the following sequence is exact
0 -> Hq{G/H,AH) lH Hq(G,A) r4s Hq(H,A).
Proof. We prove the result by induction on q. Consider the sequence
A2.1) 0 -> H1 (G/H, AH) H H1 (G, A) r4s H1 (H, A).
That inf is a monomorphism and image of inf is contained in the kernel
of res has already been proved in Theorem 11.9.1. Let / : G —> A be a
cocycle such that there exists a ? A satisfying f(h) = ha — a for every
he H. Define g : G -> A by
g(x) = f(x) — xa + a. x ? G.
Then g is a cocycle with g(h) = 0 for all h G H. For x ? G. h, ? H,
g(xh) = xg(h) + g(x) = g(x)
and
hg(x) = g(hx) — g(h) = g(hx) = g(xx~1hx) = g(x).
as x~lhx ? H. Therefore g takes values in AH. Since g(xh) = g(x) for
every x ? G. h ? H. g induces a map g' : G/H -»¦ AH by g'(xH) =
g(x). x ? G. The map g' is a cocycle and it is clear that inf ([g1]) =
[g] = [/]. This proves that Ker(res) C Im(inf) and the sequence A2.1)
is exact.
Suppose that q>2. Let B = Hom(ZG,A) be the coinduced G-module
associated with A and a : A —> B be the G-monomorphism defined by
a(a) = fa where /a(A) = Xa. A ? ZG. Identify A with the submodule
a(A) of B and set C = B/A. We have exact sequence of G-modules
A2.2) 0->A->/?->f7->0.
Since ZG is ZH-free. ZG can be written as ZH $5 M for some Abelian
group M and
B = Hom(ZG, A) = Hom(ZH ® M, A) ^ Hom(ZH, Hom(M, A)).
Therefore i? is a coinduced ff-module. Since i?J (i?, A) = 0. initial part of
the long exact sequence for cohomology corresponding to the exact sequence
A2.2) gives an exact sequence
0 -> H°(H, A) -> H°(H,B) -> H°(H, C) -> 0
or
A2.3)
© 2003 by CRC Press LLC
in which BH ^ HomH(ZG, A) ^ Hom(ZG/H, A) is coinduced as a G/H-
module. Consider the diagram
\5 \5 \5
I I I
A2.4) Hi(G/H,BH) — Hi(G,B) — H«(H,B)
which is commutative with exact columns. As /? is coinduced as a G-
module as well as -ff-module. Bff is coinduced as a G/iJ-module and
q>2,Hr(G/H,BH) = 0,Hr(G,B) =0 and Hr(H,B) = 0 for r = g-l; <7-
Therefore all the vertical maps E are isomorphisms. The long exact sequence
H{(H,A) -> H{(H,B) -> H\H,C) -> Hi+1(H,A)
has ^(ff, B) = 0 = ffi+1 (#, A) for 1 < j < g - 2. Therefore ^(ff, C) = 0
for 1 < i < q — 2. Induction hypothesis then shows that the second row
in the diagram A2.4) is exact. The exactness of the third row in this
diagram then follows by simple diagram chasing using that the maps S's
are isomorphisms. This completes induction, q
12.2 Outer Automorphisms of p-groups
One of the many important applications of homological methods to purely
group theoretic problems is the proof by Gasdmtz that every non-Abelian
finite p-group has an outer automorphism of order a power of p. Our aim
in this section is to prove this result.
Theorem 12.2.1 Let G be a finite p-group and A a G-module which is
also a finite p-group. If H1(G,A) = 0, then Hk(H,A) = 0 for all k > 1
all subgroups H of G.
Proof. We prove the result by induction on the order |G| of the group G.
Tf |G| = 1, the result follows trivially. Suppose that |G| ^ 1 and consider a
maximal subgroup Go of G. Since every maximal subgroup of a nilpotent
group is normal in the group. Go is normal in G. The five term exact
sequence
0 -> H1 (G/Go,AGo)^ Hl(G, A) -> H1 (Go, A)G
-> H2(G/Go,AGo)^ H2(G, A)
© 2003 by CRC Press LLC
along with the hypothesis Hl(G, A) = 0 shows that H^G/Go, AG°) = 0.
The subgroup Go being maximal in G. G/Gq is a finite cyclic group and
AG° is also finite (hypothesis). Therefore, the Herbrand quotient
h(Aa°) = IH^G/Go^^l/lH^G/GoiA00) = 1
and we get H2(G/G0,AGa) = 0. Hence, Hn(G/G0,AGa) = 0 for all n >
1. The above exact sequence then implies that Hl(Go,A)G = 0. Now
H1(Gq, A) is a finite G-module and G is a finite p-group. Therefore
\H1{G0,A)\ = \Hl{G{),A)G\ (modp)
and we get Hl{G0,A) = 0.
Since |Go| < \G\, the induction hypothesis shows that
A2.5) Hn(H,A) = 0 for all subgroups H of Go and all n > 1.
In particular Hn(G0,A) = 0 for all n > 1.
Let n > 2. The exact sequence
0 -> Hn(G/G0, AGo) -> Hn(G, A) -> Hn(G0, A)
then shows that Hn(G, A) = 0.
Let H be a subgroup of G and Go be a maximal subgroup of ?? such
that H C Go. It follows from A2.5) that Hn(H, A) = 0 for all n > 1. n
Lemma 12.2.2 //A is a maximal Abelian norm.al subgroup of a nilpotent
group G, then A = Co (A) - the centralizer of A in G
Proof. Let G = CG(A). Since A is Abelian. A C G. Suppose that
A < G. Define Co = G and inductively Cu+i = [Ck, G\. Since A is normal
in G, C is a normal subgroup of G and we get a decreasing sequence
Co > d > C2 > ¦ ¦ ¦ > Ck > Ck+1 >¦¦¦
of normal subgroups of G. Since G is nilpotent. there exists an rn such
that Cm+i = 1 so that Cm+i < A. Let k be the first non-negative integer
such that Ck+i < A. Then Ck ? A. Let x G Ck such that x <? A. Take
B =< x,A > - the subgroup of G generated by x and A. Since x ? Ck < C.
B is an Abelian group. For any y ? G. y~1xy = x(x~1y~1xy) which is in
< x, Ck+i >< < x, A > = B. From this it follows that B is a normal
subgroup and. so. an Abelian normal subgroup of G with A < B which is
a contradiction. Hence C = Co (A) = A. q
Theorem 12.2.3 // G is a non-Abelian finite p-group, p a prime, then p
divides \Aut (G)/Inn (G)\.
We complete the proof of the theorem in a number of steps.
© 2003 by CRC Press LLC
Lemma 12.2.4 If H is a maximal subgroup of G with C,(H) < (,{G), where
C(H). C(Gr) denote the centre of H, G respectively, then Theorem 2.3 holds.
Proof. Since every maximal subgroup of a nilpotent group is normal in
the group. H is normal in G and G/H is of order p. Every p-group has
non-trivial centre. Therefore ((H) has an element of order p. We can then
choose a homornorphism / : G —> ((H) with Ker f = H. Define a map
0 : G -> G by
cj>(x)=f(x)x, xGG.
Since f(x) G C(^) < C(Gr): <t> *s an endomorphism of G. If f(x)x = 1.
then /(/(x)x) = 1 or /(/(x))/(x) = 1 or f(x) = L as /(x) efl = ifer/.
But then x = 1 as well. Hence 0 is a monomorphism and. therefore,
automorphism of G. For any x G G.
</J(x) = </)(/(x)x) = </)(/(x)H(x) = /(/(x))/(x)/(x)x = /(xJx.
A simple induction on k shows that <f)k(x) = f(x)kx. In particular cf>p(x) =
f(x)px = x for every x ? G. Thus <fi ? Aut (G) is of order p.
Tf <fi is in Inn (G) dertermined by an element g ? G. then g ? G =
Cg{H) (because <fi fixes the elements of H in view of H = Ker f).
Case (i) If C ?H., G = HC and G/H = HC/H =s C/(H n C) = C/C(#).
Since G/H is cyclic, the group C is Abelian. In particular g commutes
with all elements of C. Already g commutes with all the elements of H.
Therefore g G C,{G).
Case (ii) If G < H, then G = ((H) < ((G) and g G ?(G).
Thus in either case g G C(Gr) anfi 0 is the identity map which is not the
ca,se. Hence <fi is indeed an outer automorphism of G. This completes the
proof, q
Let A be a maximal Abelian normal subgroup of the group G. Then
under conjugation by the elements of G. A becomes a G/A-module.
Lemma 12.2.5 If H1 (G/A, A) ^ 0, then Theorem 2.3 holds.
Proof. Let D be the group of all automorphisms of G fixing A and G/A
pointwise and -Do be the group of inner automorphisms of G denned by the
elements of A. Elements of A as well as elements of G/A are left invariant
by the elements of Dq. Therefore Dq is a subgroup of D. Let /3 be an inner
automorphism of G determined by an element x G G. If /3 leaves invariant
the elements of A, then a = xa,x~l for every a G A and x G Cg{A) = A
(by Lemma 2.2). It then follows that Do = D Pi Inn (G). Now (Theorem
11.4.7)
Q^Hl(G/A,A) ^ DI'Do = D /\D n Inn (G),
shows that there exists a 7 G D. 7 ^ Inn (G). Also H1(G/A,A) is a finite
p-group (Corollary 11.8.12) implies that order of 7 is a power of p. q
© 2003 by CRC Press LLC
Let H be the Dihedral group of order 8. Then
H=<a, b | a4 = b2 = 1, b~lab = a3 > .
The map a : H —> H which maps a to a and b to ab is an endomorphism
of H which is one-one. If a were an inner automorphism of H determined
by z ? H. then
a = a(a) = z~laz and ab — a(b) = z~lbz.
The only elements of H which commute with a are 1. a. a2. a3. However.
a~xba ^ ab. a~3ba3 ^ ab and a~2ba2 ^ ab. Hence there is no z ? H.
z ^ 1 for which z~laz = a and ab = z~lbz. Therefore a is an outer
automorphism of H. Now a2(b) = a(ab) = a?b ^ b. a3(b) = a(a2b) =
a3b ^ b but a4(&) = a(a3b) = aAb = b. Therefore a is an element of order
4. Since a~lba = a2b = a2(b) and a~laa = a. a2 is an inner automorphism
determined by a. This proves that ainn (H) is an element of order 2 in
Aut(H)/Inn(H) and, so; 2 divides \Aut(H)/Inn(H)\.
Completion of the proof of Theorem 2.3
In view of Lemmas 2.4 and 2.5 we assume that
A2.6) H1 (G/A, A) = 0 for every maximal Abelian normal
subgroup of G\
A2.7) For every maximal subgroup H of G. (,{H) ¦?. C,{G).
Take any maximal Abelian normal subgroup A of G. By A2.6) and
Theorem 2.1. H2(G/A, A) = 0. Then every extension of A by G/A splits.
In particular, the extension
1 -> A -> G -> G/A -> 1
splits and there exists a complement L of A in G i.e. L is a subgroup of
G with G = AL and ifll = {1}. Let M be a maximal subgroup of G
containing L. Then M is normal in G and G = AM. If C,{M) < Af]M = B
(say), then ((M) < ?(G) which is a contradiction to A2.7). Therefore
C(M) ? R. Then ((M)B/B ^ ((M)/(B n C(M)) ^ 1 and there exists a
subgroup R of C(M) such that R/(Bn((M)) is of order p. Set 5 = AR/A.
By Theorem 2.1. H2(S, A) = 0 which implies that As = N(A) (N is the
trace map for S) (cf. 11.2 (a)). Since R C ?(M). B = A n M C As.
Tf ,4s = A. the commutator subgroup [A,R] = 1 which implies that i? C
CG(A) = A (Lemma 2.2). This means that R C An ((M) which is not
the case. Therefore As ^ A and A/As is a non-trivial homomorphic image
of A/B = A/(A n M) = AM/M = G/M which is a cyclic group of order
p (the subgroup M being maximal). Therefore B = As. Observe that
© 2003 by CRC Press LLC
R Q C(M) implies that 5 = AR/A operates trivially oninM = B. If
A =< x,B >, then
B = AS =NS(A) =<Ns(x),Ns(B) > = <Ns(x),Bp > = < Ns(x) >
because Bv is contained in the Frattini subgroup of B.
We claim that not all Abelian normal subgroups of G are cyclic. Suppose
to the contrary that all Abelian normal subgroups of G are cyclic. Then A
and C(M) = Z (say) are cyclic. Since G is the semidirect product A ]L of
A by L and L<M,M = (AnM)]L = B]L. IfBn((M) = G (say), then
Z = ((M) = C ](C(M) r\L) = C(B (C(M) r\L)=C(B(Zr\L)
and both C and Z P\ L are non-trivial. This contradicts the assumption
that Z is cyclic. Since B is nilpotent, there exists a positive integer k such
that [Bk+i, M] < G but [Bk,M] ^ C, where Bi denotes the ith term in the
lower central series of B. Also C is normal in G. Therefore, we can choose a
y G B.y g C such that [y, M] < C. It follows that K =< y, C > is normal
in G and, so, KZ is normal in G. Also since y commutes with C elementwise
and G is Abelian, K is Abelian. Therefore KZ is Abelian and because of
the hypothesis, it is cyclic. However, (K/C) n (Z/C) = (K n Z)/C = 1 as
y(?C. Both K/C, Z/C are non-trivial. Therefore KZ/C ^ K/C 0 Z/C
is not cyclic. This contradiction, then, proves that not all Abelian normal
subgroups of G are cyclic. We can, therefore, assume that the Abelian
normal subgroup A chosen above is not cyclic. BecauseA/Z? is cyclic and
A is not cyclic, for the element x ? G already chosen, i.e., for which A =<
x,B >, we get A = B(B < x >, where order of x is p. Let s generate the
cyclic group S = RA/A. Then xs = xy, for some y ? A fl R < B. Since
S operates trivially on B and ,sp = 1, x = xs = xyp which shows that
yp = l. Then
Ns(x) = zV+2+-+(p-i) = j,p(p-i)/2.
But B =< Ns(x) > = < yPiP-1)/2 >. If p were odd, then B = 1 and
A =< x > is cyclic. This contradicts the choice of A. Hence p = 2,
B =< y > is of order 2 and A is of order 4. Tn view of the fact that G is
finite p-group having a maximal Abelian normal subgroup which is of order
p2, it follows from the theory of finite p-groups that order of G is 8. If G
were the quaternion group of order 8, then
G =< a, b | a4 = 1, a2 = b2, b~lab = a3 > .
In this G, a2 and n?b are the only elements of order 2 but the Abelian
subgroup < a2 > © < n?b > is not normal. Hence G is the dihedral
group of order 8 and for this group we have already proved that 2 divides
Aut (G)/Inn (G)\. The proof of Theorem 2.3 is now complete, q
12.2.6 Exercises
© 2003 by CRC Press LLC
1. Prove that every maximal subgroup of a nilpotent group is a normal
subgroup.
2. Tf a G-module B is finite as a group and G is finite p-group. then
\B\ = \BG\ (modp).
3. For the quaternion group G = < a. b | a4 = 1. a,2 = b2. b~1ab =
a3 > and the subgroup A = < a > of G. prove that H1 (G/A, A) is a cyclic
group of order 2.
12.3 A Theorem of Magnus
In this section our main concern is homological proof of a theorem of Mag-
Magnus given by Stammbach.
Definition 12.3.1 By the torsion free rank ro(G) of an Abelian group
G we mean the cardinality of a maximal independent subset of elements of
infinite order. In the case of a finitely generated Abelian group this is equal
to the number of infinite cyclic summands in the decomposition of G as a
direct sum of cyclic groups.
For a group G. let d(G) denote the minimum number of generators of
G.
Lemma 12.3.2 // G is a finitely generated Abelian group and ro(G) =
d(G), then G is free Abelian.
Proof. Observe that for a finitely generated torsion free Abelian group
K. ro(K) = d(K). Let H be the torsion group of G and K a torsion
free subgroup of G such that G = H ®K. Then d(G) = d(H) + d(K) =
d(H) + ro(K) = d(H) + ro(G) = d(H) + d(G). Therefore d(H) = 0 or that
H = 1 and G = K is a free Abelian group, q
A group G is said to be finitely presented if there is a free presentation
G = F/R of G in which F is a free group of finite rank and R as a normal
subgroup of F is generated by a finite number of elements.
Definition 12.3.3 Let G be a finitely presented group with a free presen-
presentation G = F/R with n generators and r relations i.e. F is a free group
of rank n and R as a normal subgroup of F is generated by r elements.
Then the number n — r is called the deficiency of the presentation
G = F/R of G. There can be several finite presentations of G and each
presentation has a deficiency. The maximum among all the deficiencies of
these presentations is called the deficiency of G and is denoted by def G.
The following result of P. Hall shows that the deficiency of a finitely
presented group is finite.
We write G' for the derived group of G and also Gi for the ith term in
the lower central series of G with G\ = G.
© 2003 by CRC Press LLC
Theorem 12.3.4 If G is a finitely presented group, then the multiplicator
M(G) (= H2G) is finitely generated and
defG<ro(G/G')-d(M(G)).
Proof. Observe that a finitely presented group is always finitely gener-
generated. For. let 1 —> R —> F ^ G —>¦ 1 be a free presentation of G with n
generators and r relations. Let {x\, • ¦ •, xn} be a set of free generators of
F. Then G is generated by xiR, • ¦ •, xnR. Also R/[F, R] can be generated
by r elements and we have d(R/[F, R\) < r. Tn the split exact sequence
1 -> R n F'/[F, R] -> i?/[F, i?] -> i?/i? n F' ^ i?F'/F' -> L
RDF'/[F, R] being subgroup of a finitely generated Abelian group is finitely
generated. But R.nF'/[F,R] ^ M(G)(= H2G) (Hopf formula). Thus
M(G) is finitely generated. Also
d(R/[F, R\) = d(R n F'/[F, R\) + d(RF'/F').
The exact sequence
1 -> i?F'/F' -> F/F' -> F/i?F' ^ G/G" -> 1
shows that
ro(F/F') = ro(RF'/F') + ro(G/G') or n = ro(RF'/F') + ro(G/G').
Therefore
r > d(R/[F, R\) = d(M(G)) + ro(RF'/F')
= d(M(G))+n-ro(G/G')
or n-r < ro(G/G') - d(M(G)).
Since this is true for every presentation and the right hand side is indepen-
independent of the presentation
defG < ro(G/G') - d(M(G)). n
We now come to the main theorem of this section. We recall the follow-
following result of Magnus
Theorem 12.3.5 If F is a free group, then fliFj = 1.
Theorem 12.3.6 Let G be a group having a presentation with n + r gen-
generators and r relators . If G/G' can be generated by n elements, say
x\G',- • •, xnG', then x\, ¦ ¦ ¦, xn generate a free subgroup of G of rank n
and, the elements xi, ¦ ¦ ¦ ,xn form a set of free qenerators.
© 2003 by CRC Press LLC
Proof. By Theorem 3.4.
n + r-r<defG< ro(G/G') - d(M(G)) <n- d(M(G))
which shows that d(M(G)) = 0 and ro(G/G') = n. Therefore M(G) =
0 and G/G' is a free Abelian group of rank n (this is so because n =
ro(G/G') < d(G/G') < n so that ro(G/G') = n = d{G/G')).
Let F be a free group with a set \yi,- • ¦ ,yn} of free generators and
8 : F —> G be a homomorphism which on the basis elements is given by
8(yi) = xi: 1 < i < n. The homomorphism 8 maps Ft into Gt for every
1 > 1 and. therefore, induces homomorphisms
8 :
/or i > 1.
Since G/G" is generated by xiG', • • • ,xnG', the homomorphism 0 induces
an epimorphism 8 : F/F' -> G/G'. Since both F/F' and G/G' are free
Abelian of the same rank n, 8 : F/F' —> G/G' is an isomorphism.
Suppose that 8 induces an isomorphism from F/Fi+\ onto G/Gi+\.
Consider the commutative diagram
j+1
A2.8)
1
'i+1
G
G/Gn
The exact sequence of Theorem 11.9.1 being natural, the same applied to
the rows in the above commutative diagram gives a commutative diagram
Hom(G/Gi+1,T)
0 » Hom(F/Fi+1,T)
— H2(G/Gi+1,T)
H2(F/Fi+1,T)
Hom(G,T)
~HomG(Gi+1,T)
Hom{F,T)
H2(G,T) = 0
H2{F,T) = 0
a
with exact rows. (Here T = Q/Z-the additive group of rationals mod 1
regarded as a trivial G-module.) For any homomorphism / : G -»¦ T.
f(a~1b~1ab) = 0 for all a. b ? G. Therefore the restriction homomorphism
: Hom(G,T) -> HomG(Gi+l,T) and Hom(F,T) -> HomF(Fi+1,T) are
the zero maps. Therefore the above diagram yields a commutative diagram
© 2003 by CRC Press LLC
0
Hom(Gi+1/Gl+2,T)
Hom(Fl+1/Fi+2,T)
H2(G/Gi+1,T)
H2(F/Fi+1,T)
0
with exact rows. Since 9 : F/F{+i —> G/Gi+i is an isomorphism, so is the
induced homomorphism 9* : H2(G/Gi+1,T) -> H2(F/Fi+1,T). Therefore
9 induces an isomorphism
0* : Hom(Gl+1/Gi+2,T) -> Hom(Fi+1/Fi+2,T).
Let A be the kernel of the homomorphism 9 : ^+1/^+2 —> Gj+i/Gi+2 anfi
B be the cokernel of this map. The functor Hom(—,T) being exact (since
T is an injective Z-module) we get exact sequences
Hom(Gi+1/Gi+2,T) ^ Hom(Fi+1/Fi+2,T) -> Hom(A,T) -> 0;
0 -> Hom(B,T) -> Hom(Gi+1/Gi+2,T) ^ Hom(Fi+1/Fi+2,T).
The map ^* being an isomorphism we get Hom(A,T) = Hom(B,T) = 0.
These then imply that A = B = 1. Hence 8 : Fi+i/Fi+2 -»¦ Gi+i/Gi+2 is
an isomorphism. In the commutative diagram
l /Fi+2
A2.9)
G/G
i+2
G/Gt
+1
the two vertical maps on the left and on the right are isomorphisms. An easy
diagram chasing (the groups involved are not all Abelian) then shows that.
6 : F/Fi+2 ~^ G/Gi+2 is also an isomorphism. This completes induction
and, hence, 9 : F/Fi+i —> G/Gj+i is an isomorphism for alii > 1. Therefore
Ker F: F^G) < Fi+1 for all i > 1 or that Ker F : F -> G) < niFi+1 =
1. Hence 9 is an embedding and image of 9 which is generated by x\, • ¦ •, xn
is a free group of rank n freely generated by these elements, q
Corollary 12.3.7 Let G be a group having a presentation with n + r gen-
generators and r relators. If G can also be generated by n elements, then G is
a free group of rank n.
Proof. Tf x\, ¦ • ¦,xn generate G, then X\G',- • •,xnG' generate G/G'.
The result then follows from Theorem 3.6. q
Proceeding on the lines of the proof of Theorem 3.6, we can also prove
the following :
© 2003 by CRC Press LLC
Theorem 12.3.8 Let G be a group and p be a prime numher such that
H-2(G,ZP) = 0. Let {xa} be a set of elements of G such that the set of
elements {xaG'Gp} is linearly independent over the field Zp. Then {xa} is
a basis of a free subgroup of G.
Theorem 12.3.9 Let G be a group such that H2(G,Q) = 0, where Q is
the field of rational numbers. Let {xa} be a set of elements of G such that
the set {xaG' <g> 1} of elements of (G/G1) ® Q are linearly independent over
Q. Then {xa} is a basis of a free subgroup of G.
12.3.10 Exercises
1. Let G be a finitely generated Abelian group generated by x\, ¦ ¦ ¦, xn.
Tf ei, ¦ • ¦, en are non-negative integers with x^1 • ¦ ¦ xf{1 = 1 and ei, • • •, en
are relatively coprime. prove that G can be generated by n — 1 elements.
2. Let G =< X\, ¦ • ¦, xn | u > where u is a word in x\, • • •, xn such that,
sum of all exponents of Xi in u is e^. 1 < i < n. If g.c.d.(ei, ¦ ¦ ¦ ,en) = 1.
prove that G is a free group of rank n — 1.
3. Tf G is an Abelian group and m is a positive integer, prove that
G 0 Zm =s G/Gm.
4. For an Abelian group G. prove that. ro(G) is equal to the dimension
of the vector space G ® Q over the field Q of rational numbers.
5. If A is an Abelian group such that Horn (A, T) = 0, prove that A = 1.
© 2003 by CRC Press LLC
Bibliography
[I] M. Auslander, T. Reiten and S. 0. Smalo, Representation Theory of
Artin Algebras. Cambridge University Press. 1995.
[2] M. Bar and G.S. Rinehart, Cohomology as the derived functor of
derivations, Trans. Amer. Math. Soc,122A966), 416-426.
[3] F.R. Beyl and ,T. Tappe, Group Extensions. Representations and
the Sehur's Multiplicator. Lecture Notes in Mathematics No.958.
Springer- Verlag. Berlin-Heidelberg-New York. 1972.
[4] H. Cartan and S. Eilenberg, Homological Algebra. Princeton Univer-
University Press. Princeton. New Jersey,1956.
[5] P. Cartier. The groups Ext (A,B). Seminaire A. Grothendieck (Alge-
(Algebra Homologique), 1956-57.
[6] J.W.S. Cassels and A. Frohlich. Algebraic Number Theory. Academic
Press,1967.
[7] C. W. Curtis and T. Reiner, Reprentation Theory of Finite Groups
and Associative Algebras, Interscience Publishers, John Wiley and
Sons, New York, London, 1962.
[8] A. Dold, Homology of symmetric products and other functors of com-
complexes, Ann. of Math., 68A958), 54-80.
[9] B. Eckmann. P..T. Hilton and U. Stammbach, On the homology the-
theory of central group extensions, I-The commutator map and stem
extensions, Comment. Math. Helv.. 47A972),102-122.
[10] S. Eilenberg and S. Maclane, Cohomology theory of abstract groups-I,
Ann. of Math., BL8A947), 51-78.
[II] S. Eilenberg and S. Maclane, Cohomology theory in abstract groups-
II, Ann. of Math., BL8A948), 326-341.'
[12] S. Eilenberg and N. Steenrod, Foundations of Algebraic Topology,
Princeton University Press, Princeton, 1952.
© 2003 by CRC Press LLC
[13] W. Gaschiitz, Kohomologische Trivialitaten und riussere Automor-
phismen von p-Gruppen, Math. Z., 88A965). 432-433.
[14] W. Gaschiitz. Niehtabelsehe p-Gruppen besitzen missere p-
Automorphismen, J. Algebra. 4A966). 1-2.
[15] K.W. Gruenberg, Cohomological Topics in Group Theory. Lecture
Notes in Mathematics No. 143. Springer-Verlag, Berlin-Heidelberg-
New York, 1970.
[16] P. R. Halmos, Naive Set Theory. Affiliated East-West Press Pvt. Ltd..
New Delhi, 1972.
[17] P.J. Hilton and D. Rees. Natural maps of extension functors and a
theorem of R.G. Swan, Proc.Cambridge Philos. Soc.,57A961), 489-50.
[18] P.J. Hilton and U. Stammbach, A Course in Homological Algebra,
Springer-Verlag, Berlin- Heidelberg- New York,1970.
[19] G. Hochschild, Relative homological algebra, Trans. Amer. Math.
Soc, 82A956), 246-269.
[20] G. Hochschild and J.P. Serre, Cohomology of group extensions, Trans.
Amer. Math. Soc, 74A953), 110-134.
[21] N. Iwahori and H. Matsumoto, Several remarks on projective repre-
representations of groups, J. Fac. Sci., University Tokyo. 10A963-64), 129-
146.
[22] N. Jacobson, Basic Algebra I, Hindustan Publishing Corporation,
Delhi,1984.
[23] I. Kaplansky. Infinite Abelian Groups, The University of Michigan
Press, Ann. Arbor, 1965.
[24] F. Kash, Modules and Rings, Academic Press, London-New York,
1982.
[25] I. S. Luthar and I. B. S. Passi, Algebra 1 : Groups, Narosa Publishing
House, New Delhi-Madras-Bombay-Calcutta-London. 1996.
[26] R.C. Lyndon. The cohomology theory of group extensions, Duke
Math. J., 15A948), 271-292.
[27] S. Maclane, Homology, Springer-Verlag, Berlin -New York, 1963.
[28] W. Magnus, Beziehungen zwisehen Gruppen und Idealen in einem
speziellen Ring, Math. Ann., 111A935), 259-280.
[29] W. Magnus, Uber freie Factorgruppen under freie Untergruppen
gegebener Gruppen, Monatsh. Math. Phys.. 47A939), 307-317.
© 2003 by CRC Press LLC
[30] D.G. Northcott, An Introduction to Homological Algebra. Cambridge
University Press. London and New York. 1960.
[31] I.B.S. Passi, Polynominal functors. Proc.Cambridge Philos. Soc., 66
A969), 505-512.'
[32] I.B.S. Passi. Group Rings and Their Augmentation Ideals. Lecture
Notes in Mathematics No.715. Springer-Verlag, Berlin- Heidelberg-
Yew York. 1979.
[33] I.B.S. Passi. S. Sharma and L.R. Vermani, A filtration of the varietal
multiplicator. Proc. Nat. Acad. Sci. India (P.L.Bhatnagar Commem-
Commemorative volume). A979). 377-388.
[34] P. Ribenboim. Rings and Modules. Interscience Tracts in Pure and
Applied Mathematics No.6. Interscience. New York. 1969.
[35] D..T.S. Robinson. A Course in the Thoery of Groups. Springer-Verlag.
Newyork-Heidelberg-Berlin, 1982.
[36] J. Rotman. An Introduction to Homological Algebra. Academic
Press. New York. San Francisco. London. 1979.
[37] W.R. Scott, Group Theory. Printice-Hall, Inc. Eaglewood Cliffs, New
Jersey. 1964.
[38] T.G. Singh, and L.R. Vermani. On some exact sequences in the co-
homology of groups, J. Indian Math. Soc, 45A981), 359-365.
[39] J.P. Serre, Local Fields, Springer-Verlag, New York Heidelberg Lon-
London, 1979.
[40] J. Stallings, Homology and central series of groups, J. Algebra,
2A966), 170-181.
[41] U. Stammbach, Uber freie Untergruppen gegebener Gruppen, Com-
Comment. Math. Helvetici, 43A968), 132-136.
[42] U. Stammbach, Anwendungen der Homologie theorie der Gruppen
auf Zentralreihen und auf Invarianten von Prasentierungen, Math.
Z., 94A966), 157-177.
[43] U. Stammbach, Homology in Group Theory, Lecture Notes in Mathe-
Mathematics No. 359, Springer-Verlag, Berlin- Heidelberg- New York,1973.
[44] M. Suzuki, Group Theory I, Springer-Verlag, New York-Berlin-
Heidelberg-Tokyo, 1977.
[45] M. Suzuki, Group Theory II, Springer-Verlag, New York-Berlin-
Heidelberg-Tokyo, 1986.
© 2003 by CRC Press LLC
[46] L.R. Vermani, An exact sequence and a theorem of Gaschutz,
Neitmser and Yen on the multiplicator. ,T. London Math. Soc, BI
A969), 95-99.
[47] L.R. Vermani. On polynomial groups. Proc. Gamb. Philos. Soc. 68
A970), 285-289.
[48] L.R. Vermani. On the multiplicator of a finite group, J. London Math.
Soc, B)8A974), 765-768.
[49] L.R .Vermani, An exact sequence, Bull London Math. Soc, 6A974),
349- 353.
[50] L.R. Vermani, The exact sequence of Hochschild-Serre in the coho-
mology of groups, J. London Math. Soc, BI2A976), 291-297.
[51] L.R. Vermani, A Note on Yoneda product, J. Math.Soc.Japan, 33
A981), 591-594.
[52] L.R. Vermani, Lectures in Cohomology of Groups, Kurukshetra Uni-
University Press, 1994/95.
© 2003 by CRC Press LLC